Analysis of Unilateral Rail Corrugation Mechanism Based on Friction Self-Excited Theory
-
摘要:
为了分析重载铁路曲线地段钢轨波磨的产生原因,基于摩擦自激振动理论建立小半径曲线轮轨三维接触精细化模型,讨论了不同扣件刚度、摩擦系数、超高对轮轨系统不稳定摩擦自激振动的影响,揭示了单侧钢轨波磨产生的内在原因,并通过轮轨瞬态动力学方法,分析了单侧钢轨波磨的传递及演化过程. 结果表明:超高和实际运行速度的不匹配是曲线内股钢轨首先产生波磨的主要原因;内股钢轨波磨产生后会导致轮轨系统不稳定,并将振动传递至外股钢轨,从而诱发小半径曲线地段两侧钢轨均产生波磨;适当地提高扣件垂横向刚度、控制轮轨摩擦系数在0.4以下,能够有效地降低轮轨系统发生不稳定振动的趋势,从而抑制波磨发展.
Abstract:In order to analyze the causes of rail corrugation in curve section of heavy haul railway, a refined wheel-rail three-dimensional contact model is established based on the friction self-excited vibration theory. The influences of different stiffness, friction coefficient and superelevation on the unstable friction self-excited vibration of wheel-rail system were discussed, and the internal causes of single rail corrugation were revealed, and the transmission and evolution process of single rail corrugation were analyzed by means of the explicit dynamic model. The results show that the mismatch between superelevation and actual running speed is the main cause of unilateral rail corrugation. The corrugation of the inner rail will lead to the instability of the wheel/rail system, and the vibration will be transmitted to the outer rail, which will induce corrugation of the rails on both sides of the small radius curve section. By properly improving the vertical and lateral stiffness of fasteners and controlling the wheel-rail friction coefficient below 0.4, the tendency of unstable vibration of wheel-rail system can be effectively reduced, and the development of ripples can be restrained.
-
钢轨波浪形磨耗是指钢轨表面出现的周期性波浪形不平顺现象,又称作波磨[1]. 从已有文献来看,钢轨波磨在世界各国的铁路及城市轨道交通都普遍存在. 中国在重载铁路上波磨尤其严重,据统计,约40%以上的重载铁路曲线地段均存在钢轨波磨[2],特别是在小半径曲线(半径R ≤ 800 m)地段,波磨现象出现早,发展快. 由波磨引起的各种轨道结构病害也急剧增加,这些不仅增加了重载铁路养护维修工作量,也直接威胁运营安全.
钢轨波磨产生机理复杂,影响因素众多,国内外学者据此进行了大量的研究,至今未形成共识:英国学者Xie等[3]发现如果选择赫兹接触,各种磨损和法向力之间的相移近似相同,由此导致初始不平顺继续发展;国内Jin等[4-6]对初始不平顺导致钢轨波磨产生的机理进行了分析;张厚贵[7]针对北京地铁异常波磨现象进行了大量的研究,认为剪切型减振扣件导致的轨道结构阻尼过低,钢轨极易发生不稳定振动,从而导致钢轨波磨的形成;Chen等[8-9]提出了钢轨波磨的发生源于饱和蠕滑力诱发的轮轨系统摩擦自激振动;肖祥龙等[9-12]提到小半径曲线地段钢轨波磨首先出现在一侧,但是关于波磨首先出现在哪一侧尚不明确.
由上述国内外已有研究可以看出,轮轨间饱和蠕滑力引发的不稳定摩擦自激振动是导致轮轨间产生波磨的主要诱因之一. 同时,钢轨波磨的不对称性普遍存在,但这种现象的产生原因以及演化机理尚未被探讨.
基于此,本文在现场钢轨波磨调研基础上,采用饱和蠕滑力诱发轮轨系统摩擦自激振动的理论,建立精细化的轮轨三维接触有限元模型;基于等效阻尼比进行复特征值计算,分析不同扣件刚度、摩擦系数和超高等因素对轮轨系统不稳定摩擦自激振动的影响,研究单侧钢轨波磨的产生原因;进一步结合瞬态动力学方法模拟钢轨表面波磨几何特征,分析单侧钢轨波磨的传递及演化过程,揭示不对称钢轨波磨产生的原因,为养护维修提供指导.
1. 现场测试
1.1 钢轨波磨测试
神朔重载铁路是我国西煤东运大通道神黄铁路的一部分,主要开行C80、C70、C64货车,平均速度50 km/h,另外还开行一辆通勤客车,平均速度70 km/h. 为提高旅客乘坐的舒适性,在曲线地段的超高均按过超高设置. 采用钢轨波磨测量仪(CAT)在现场测试钢轨表面的粗糙度.
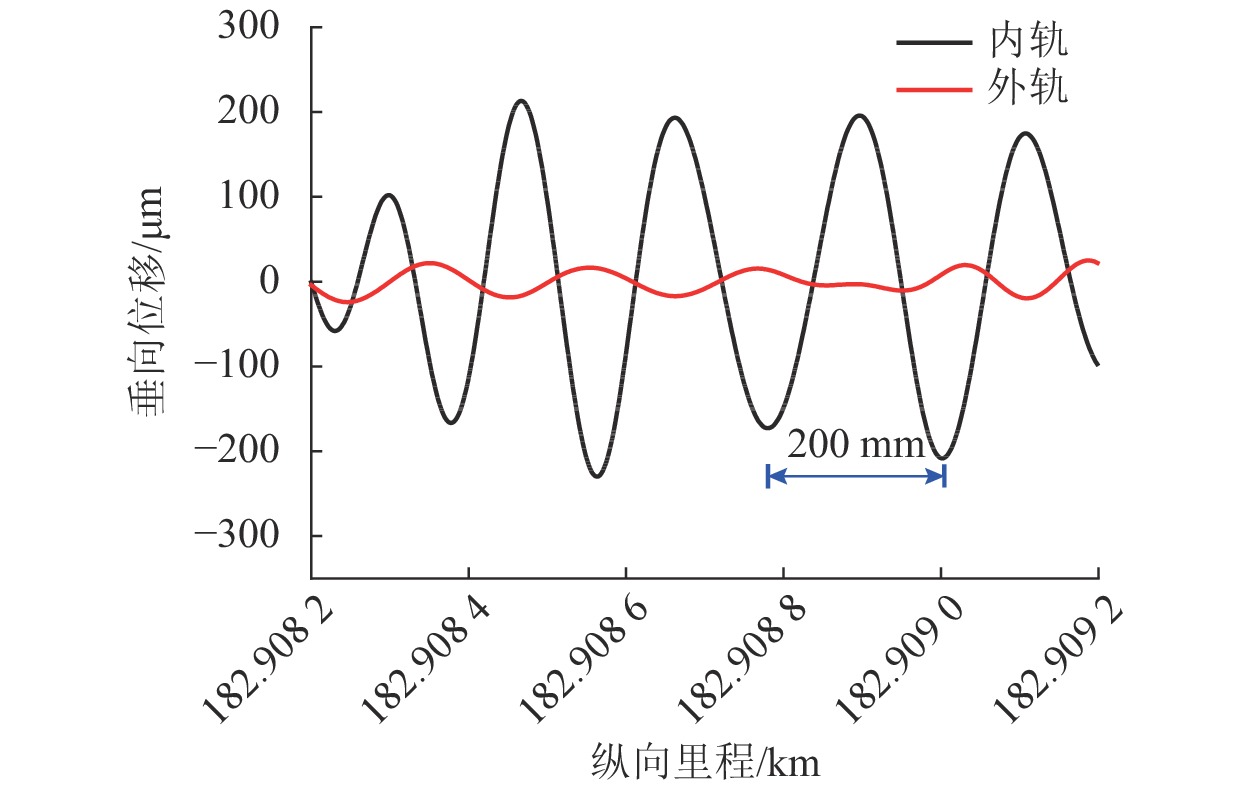
图1为用CAT钢轨波磨仪测得的钢轨表面垂向不平顺局部放大图. 测试的波磨完整图形见补充材料图S1,对应的频谱图见补充材料图S2. 从图1可以看出:内轨表面波长约为200 mm,波深约为0.5 mm,属于中波波磨不平顺,计算可知其对应的振动频率为69 Hz;外轨也检测出波长为200 mm的不平顺,但是波深较小. 此外,通过现场调研及跟踪测试发现,神朔重载铁路小半径曲线地段钢轨波磨先在内侧钢轨出现,之后随着时间的推移,另一侧钢轨表面也会产生同波长的波磨不平顺,整个过程中内侧钢轨波磨始终比外轨大.
1.2 轮轨作用力测试
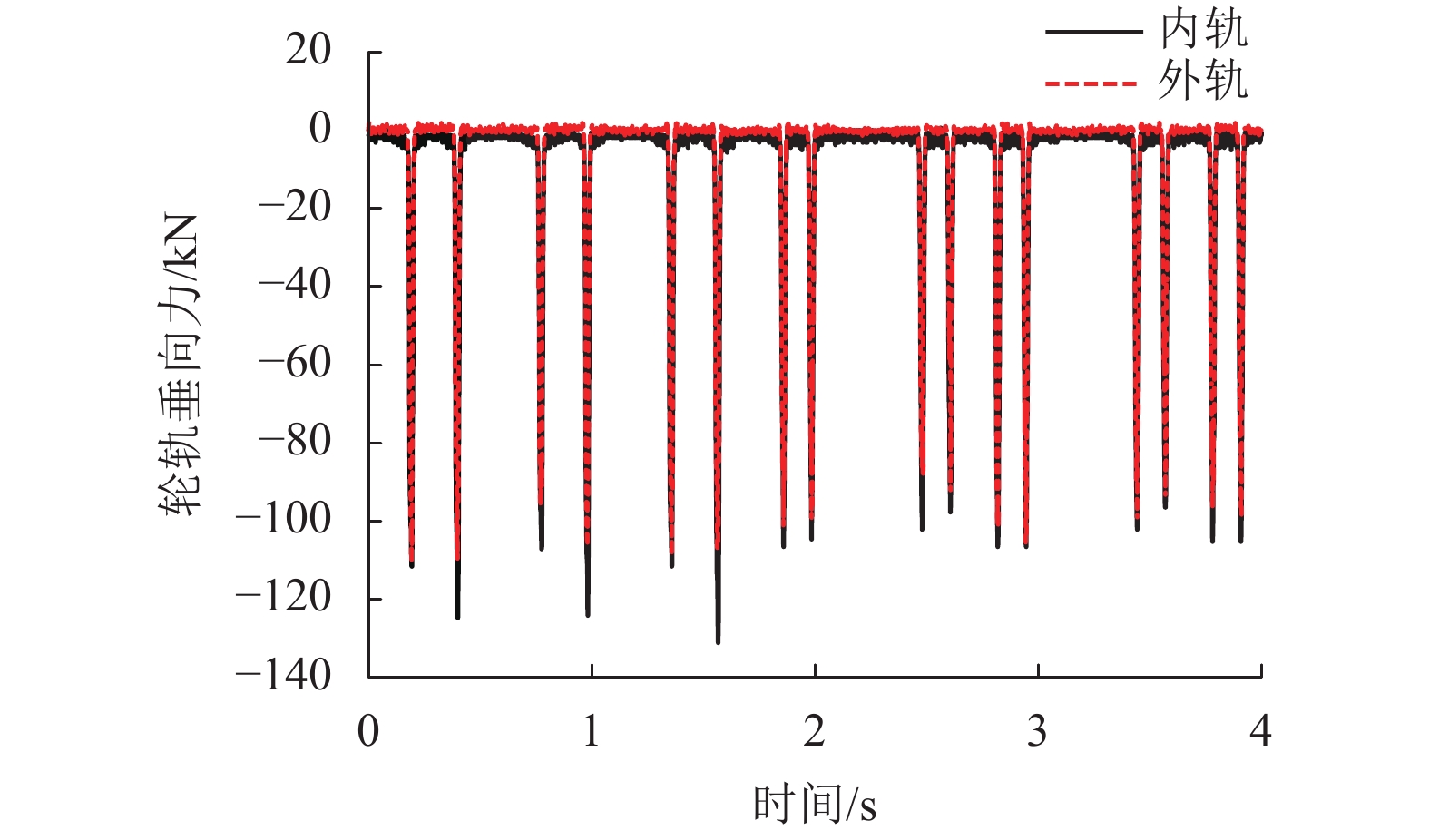
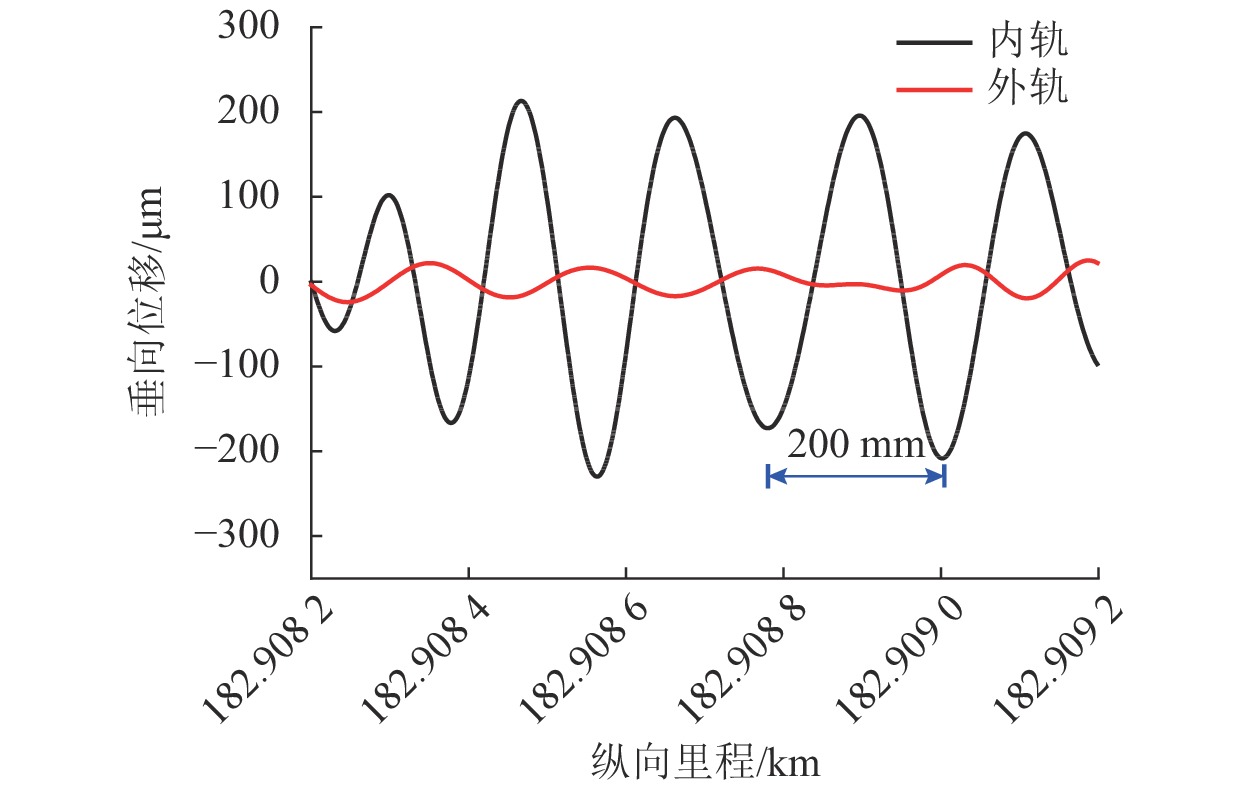
为验证后续模型,在现场对轮轨的垂向作用力进行了测试,测试结果如图2所示.
从图2可以看出:在峰值及非峰值处,外轨受力均略小于内轨,内、外轨轮轨垂向力分别为132.11 kN和126.72 kN,内轨受力较大,这主要是由于曲线地段过超高,以及内轨不平顺严重造成的. 该试验结果将用于后续模型验证.
2. 钢轨波磨仿真分析模型
2.1 轮轨摩擦自激振动及瞬态动力作用形成波磨机理
诸多学者认为,轮轨间摩擦功的变化会导致钢轨表面产生相应的磨耗与伤损[13-19],根据Brockley等[20-23]提出的轨面磨损量的计算公式以及摩擦自激振动理论,在轮轨系统摩擦自激振动的影响下,法向接触力会产生相同频率的振动,进而导致摩擦功也产生同频率的周期性波动,这几种频率均来自整个轮轨系统,进而导致单位时间内钢轨表面磨损量亦发生相应的波动,由此产生钢轨波磨,详细内容见附加材料1.
2.2 复特征值分析法
复特征值分析法是一种能够判定系统产生摩擦自激不稳定振动频率及其对应复模态主导振型的线性方法. 由于涉及弹性体之间的耦合作用,考虑摩擦的系统模型表现得非常复杂. 一般认为,高频率的工况不适用无质量弹簧方法. 这里采用无弹性接触面只约束接触节点的方法[8],详细推导过程见附加材料2. 系统对应第i阶次的等效阻尼比
ξi 可以判断系统自激振动发生的趋势,如式(1).ξi=−αi/(π|ωi|), (1) 式中:
αi 为特征值实部,i为阶次;ωi 为特征值虚部,即当ξi 为负值时,系统发生自激振动的可能性也会增大,ωi 绝对值越大,系统越容易发生不稳定自激振动.2.3 轮轨三维接触有限元模型
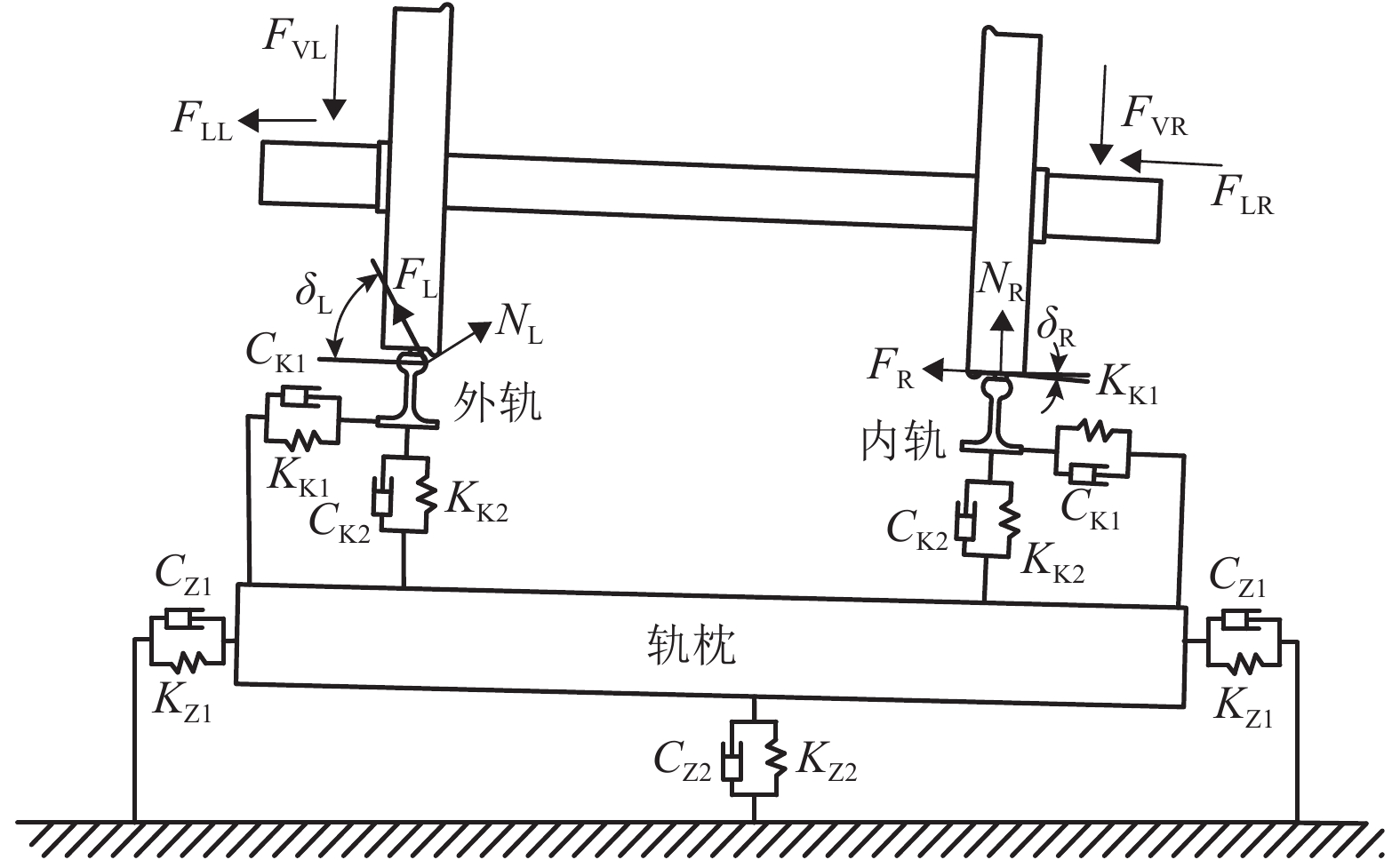
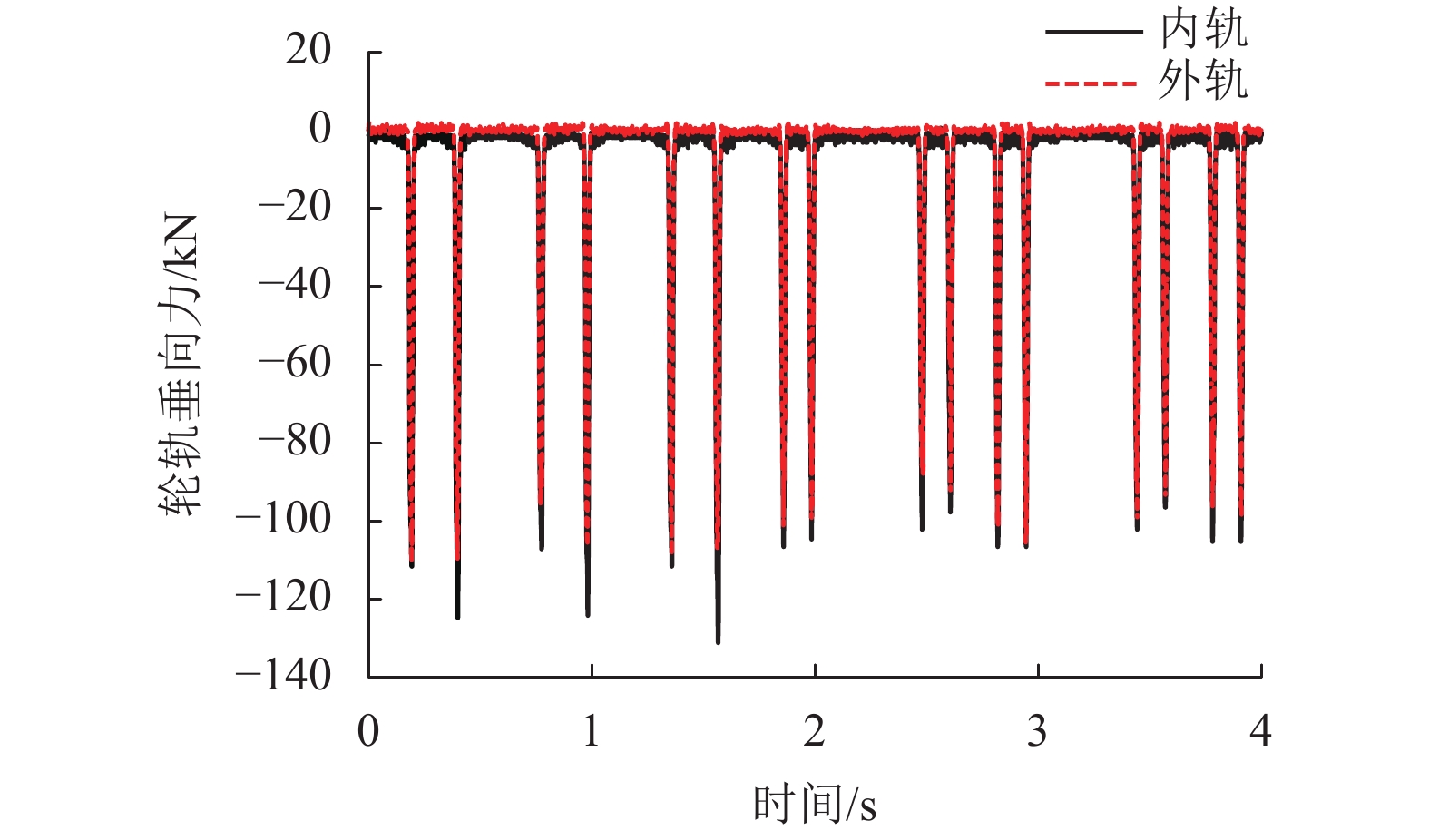
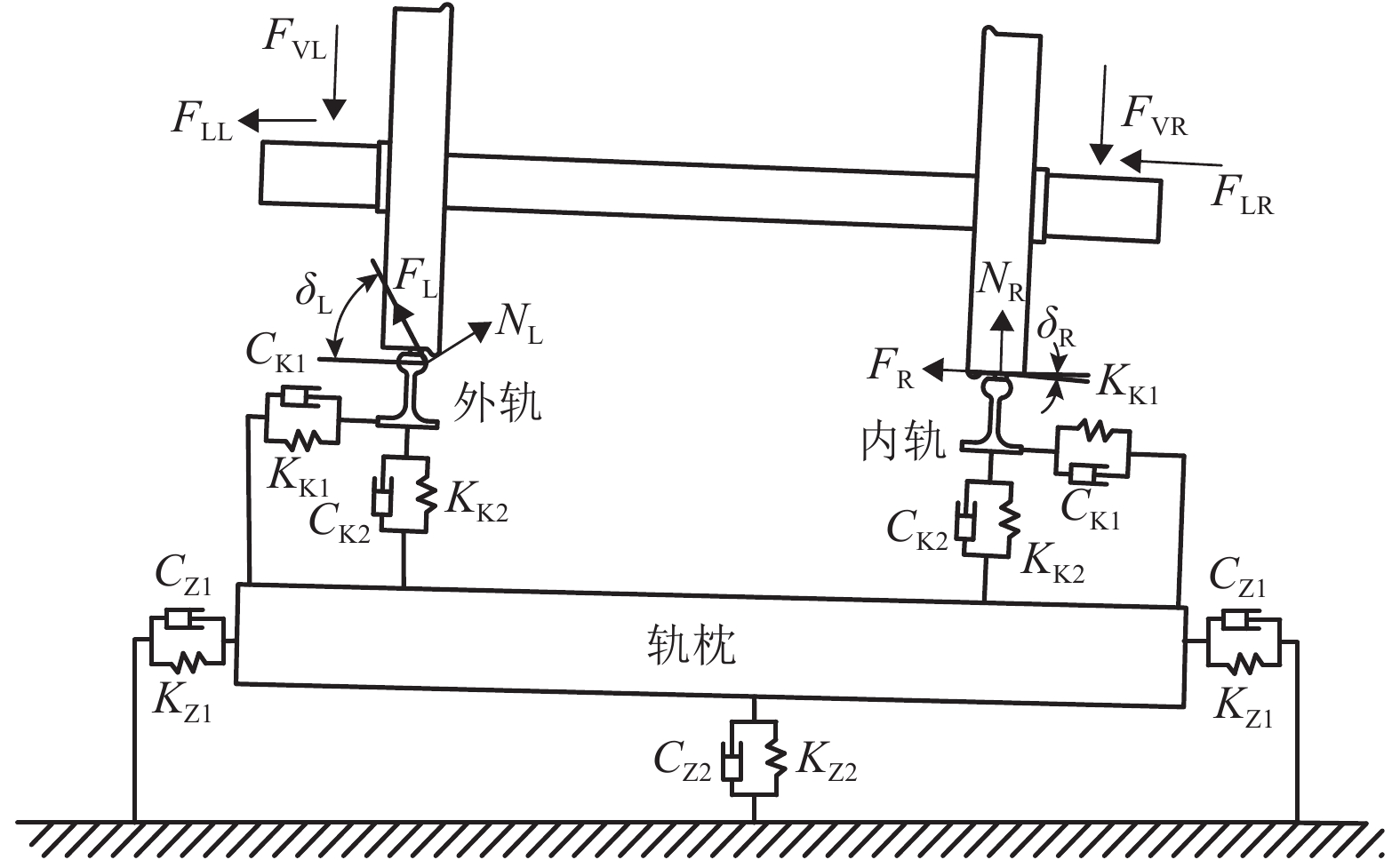
重载铁路小半径曲线段轮轨接触力学模型,通过在轮对两端施加垂、横向悬挂力以模拟悬挂系统对轮对的作用,如图3所示. 图中:
FVL 、FVR 分别为曲线外轨、内轨侧转向架作用在车轴上的垂向力;FLL 、FLR 分别为曲线外轨、内轨侧转向架作用在车轴上的横向力;NL 、NR 分别为曲线外轨、内轨侧轮轨法向力;FL 、FR 分别为曲线外轨、内轨侧轮轨横向力;δL 、δR 分别为曲线外轨、内轨侧轮轨接触角;KK1 、KK2 分别为扣件系统横向、垂向刚度;CK1 、CK2 分别为扣件系统横向、垂向阻尼;KZ1 、KZ2 分别为枕下横向、垂向刚度;CZ1 、CZ2 分别为枕下横向、垂向阻尼,其中轮轨横向蠕滑力、法向力以及接触角由多体动力学软件Simpack计算得到. 车辆选用神华号电力机车,计算工况地段线路形式为曲线,半径R = 400 m,超高105 mm,车速50 km/h. 其中,车辆参数见附加材料表S1. 材料瑞利阻尼系数β取0.0015,依此建立机车的轮轨三维接触有限元模型,其材料参数与详细模型参数设置见附加材料表S2、S3所示.由于车辆悬挂装置和减振系统的作用,在复模态分析时忽略其簧上质量的影响,着重考虑轮轨接触系统处在平衡状态时轮轨产生相对纵向滑移发生的弹性自激不稳定振动情况,在复模态分析模型中仅考虑轮对、钢轨和轨枕等主要结构. 车轮踏面采用JM3型,钢轨廓形为CHN75型. 钢轨长度取36 m,由61根Ⅲ型轨枕和扣件系统分散支撑. 利用Python脚本语言批量建立wire连接以模拟钢轨和轨枕之间的扣件系统. 模型单元类型为C3D8I,单元总数为234552个,节点总数为312609个,模型效果示意图见附加材料图S3.
由于本模型是根据重载铁路小半径曲线段现场实际线路参数以及列车车型、速度等运营参数建立的,将模型仿真计算结果与现场实测结果进行对比,如表1所示,现场轮轨力实测结果与仿真结果较为接近,所建模型可靠性较好,可用于后续的研究分析.
表 1 计算结果对比Table 1. Comparison of calculation resultskN 项目 外轨垂向力 内轨垂向力 现场实测值 126.72 132.11 模型计算值 124.66 128.86 注:现场实测值取多测点平均值,模型计算值取不平顺曲线段平均值. 3. 钢轨波磨产生原因分析
扣件刚度、轮轨摩擦系数、超高与速度等对钢轨波磨有直接影响.
3.1 扣件刚度对轮轨系统不稳定性的影响
钢轨扣件刚度是保证轨道结构安全、评价扣件性能最重要的参数之一. 应用复特征值分析法,就扣件刚度对轮轨系统摩擦自激振动的影响进行分析,从而进一步了解扣件垂、横向刚度对钢轨波磨产生的影响. 根据现场实际情况,设置如表2所示的组合工况.
由摩擦自激振动理论可知:负等效阻尼比的绝对值越大,系统越容易发生摩擦自激振动,导致钢轨波磨进一步发展;钢轨波磨的发生频率与该不稳定摩擦自激振动频率相对应;最小等效阻尼比出现的频率所对应的不稳定振型为轮轨系统的主导振型,对系统的影响最大,最容易发生不稳定振动.
由于重载铁路钢轨波磨频率范围一般在20~120 Hz[2,7,9],所以本文在复模态分析时,为了节约计算资源,只提取20~120 Hz内的模态计算结果. 9种工况的等效负阻尼比值计算结果如图4所示(红色、绿色投影部分分别表示不同扣件垂向、横向刚度下等效阻尼比的大小),由图4可知:整个轮轨系统的负等效阻尼比绝对值会随着钢轨扣件垂、横向刚度的增大而减小,说明一定程度地增大扣件垂向刚度和横向刚度能够显著地抑制自激振动,从而抑制钢轨波磨的发展.
表 2 扣件刚度计算工况Table 2. Working conditions of fastener stiffness calculationMN/m 刚度 工况 1 工况 2 工况 3 工况 4 工况 5 工况 6 工况 7 工况 8 工况 9 横向 40 40 40 60 60 60 80 80 80 垂向 40 80 120 40 80 120 40 80 120 选取具有代表性的刚度最大、最小和一般工况下负等效阻尼比分布及主导自激振动振型图,见附加材料图S4~S6,放大系数均为10000. 3种工况的最小等效阻尼比分别出现在65、70、74 Hz处,各工况的主导不稳定自激振动振型均出现在65~75 Hz范围内,与现场情况较为吻合.
同时,3种工况的最小负等效阻尼比值分别为−0.02189、−0.02101和−0.01982,变化不大. 在第一种工况下,当扣件垂向刚度和横向刚度都较小时,不稳定振动振型最为复杂. 这也反映了增大扣件垂向刚度和横向刚度,能够显著地抑制自激振动,从而抑制钢轨波磨的发展.
另外,对比振型可以发现,各工况最小负等效阻尼比对应的不稳定振动均发生在轮轨系统的内轮-内轨上,与该重载铁路小半径曲线现场波磨产生情况较为符合.
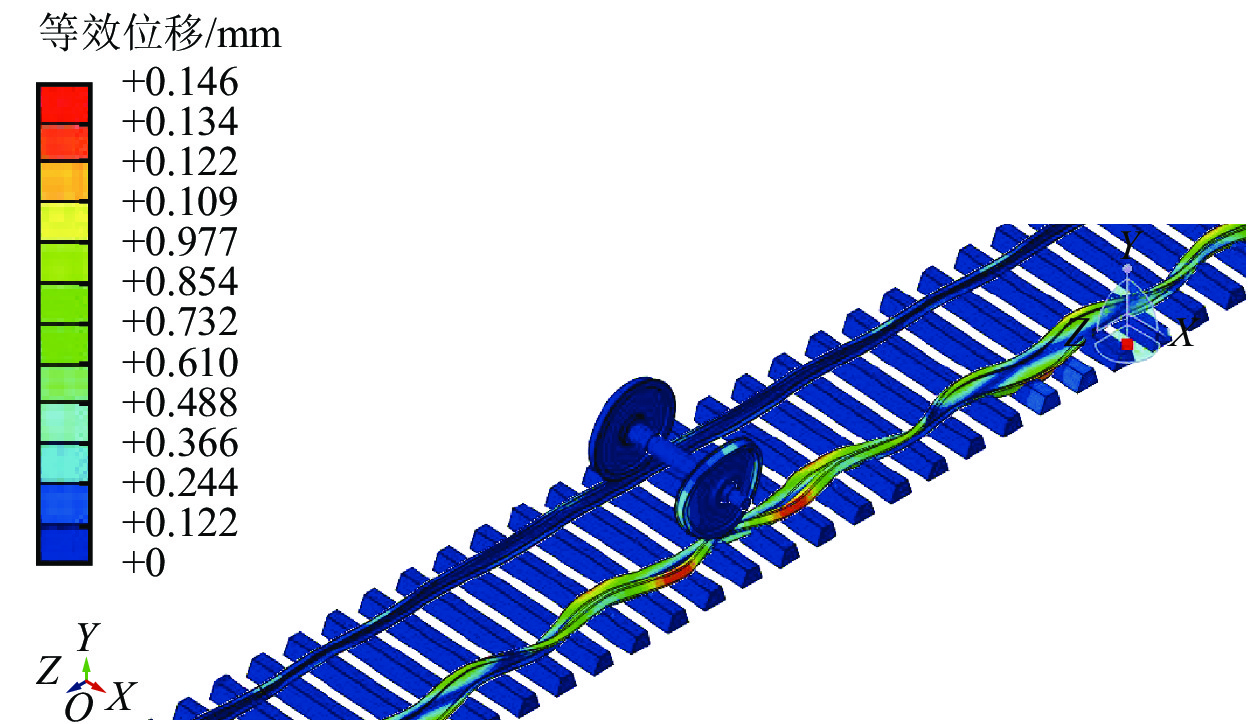
为了便于观察,附加材料图S4~S6中列出了其横断面视角,图5为工况1的主导不稳定自激振动振型对应的三维视角图. 9种工况的主导不稳定振动振型非常类似,且均发生在曲线的内轮-内轨处,故此处不再重复罗列其三维视角图.
查阅文献可知,北京地铁波磨病害现场也有类似反映[7],使用弹性扣件的线路比使用普通扣件的地段更容易产生波磨病害,这和本文的分析结果十分吻合,也从实际角度进一步证明了饱和蠕滑力会导致轮轨系统摩擦自激振动,导致波磨的发生和发展.
3.2 摩擦系数对轮轨系统不稳定性的影响
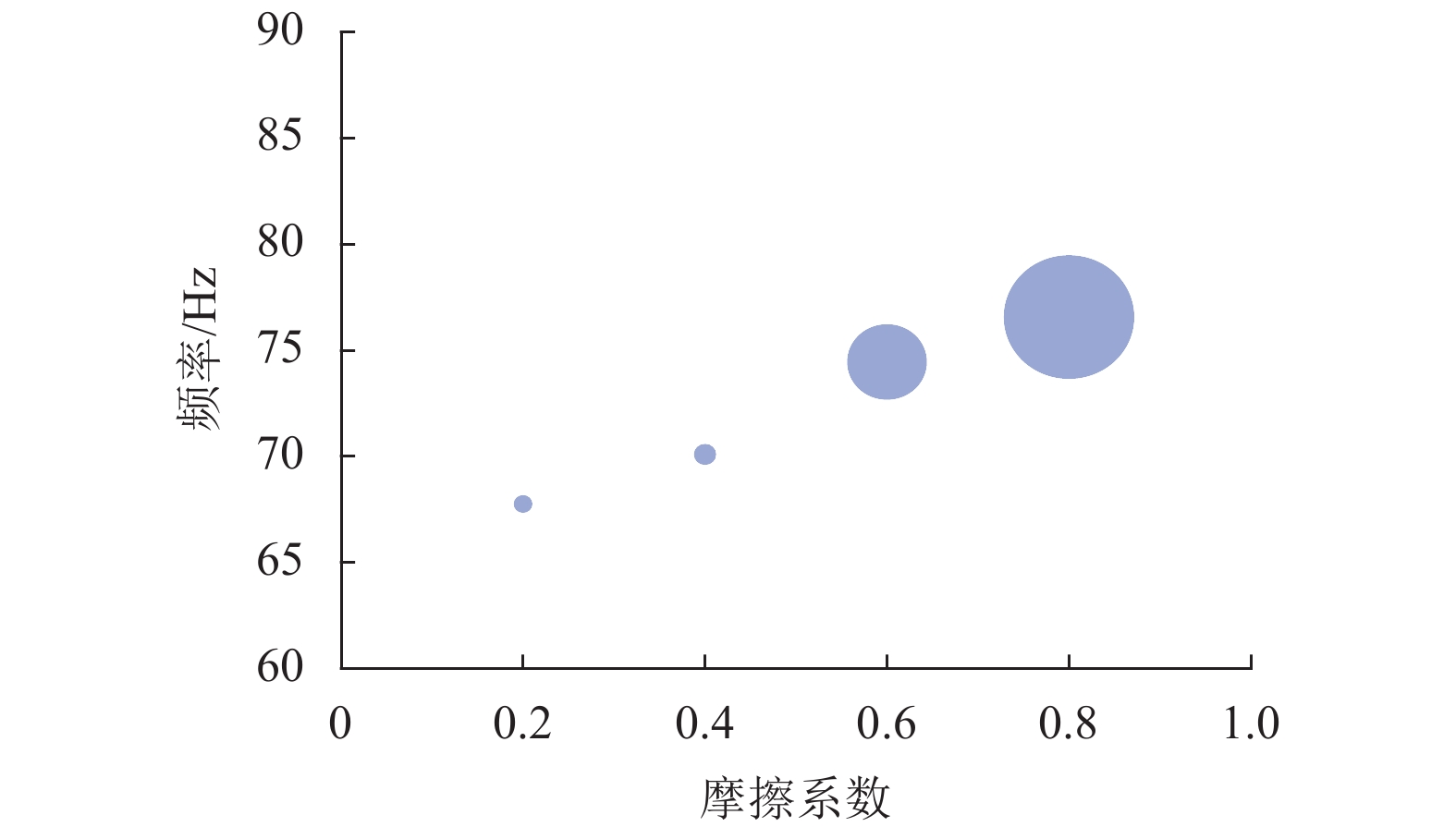
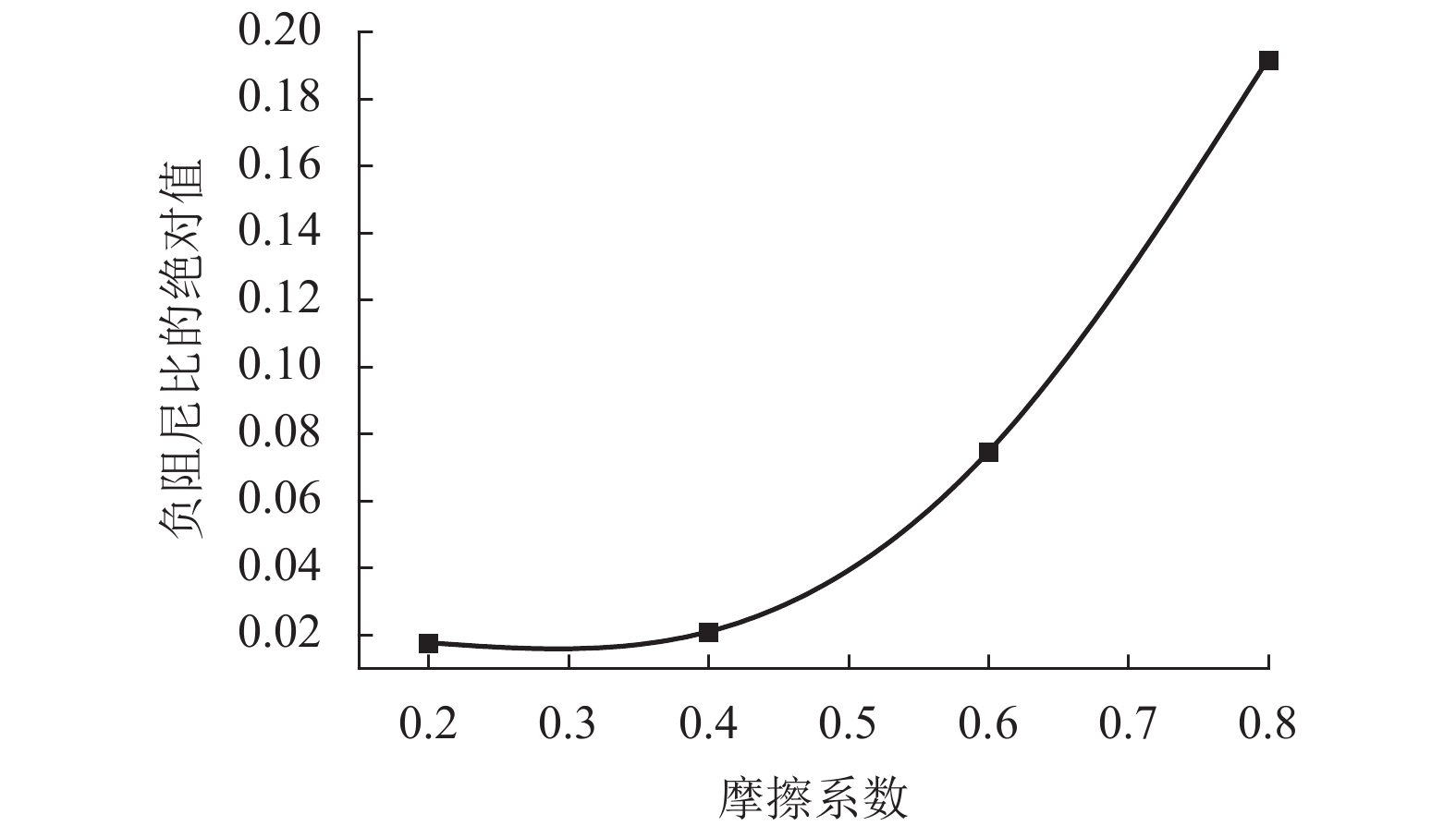
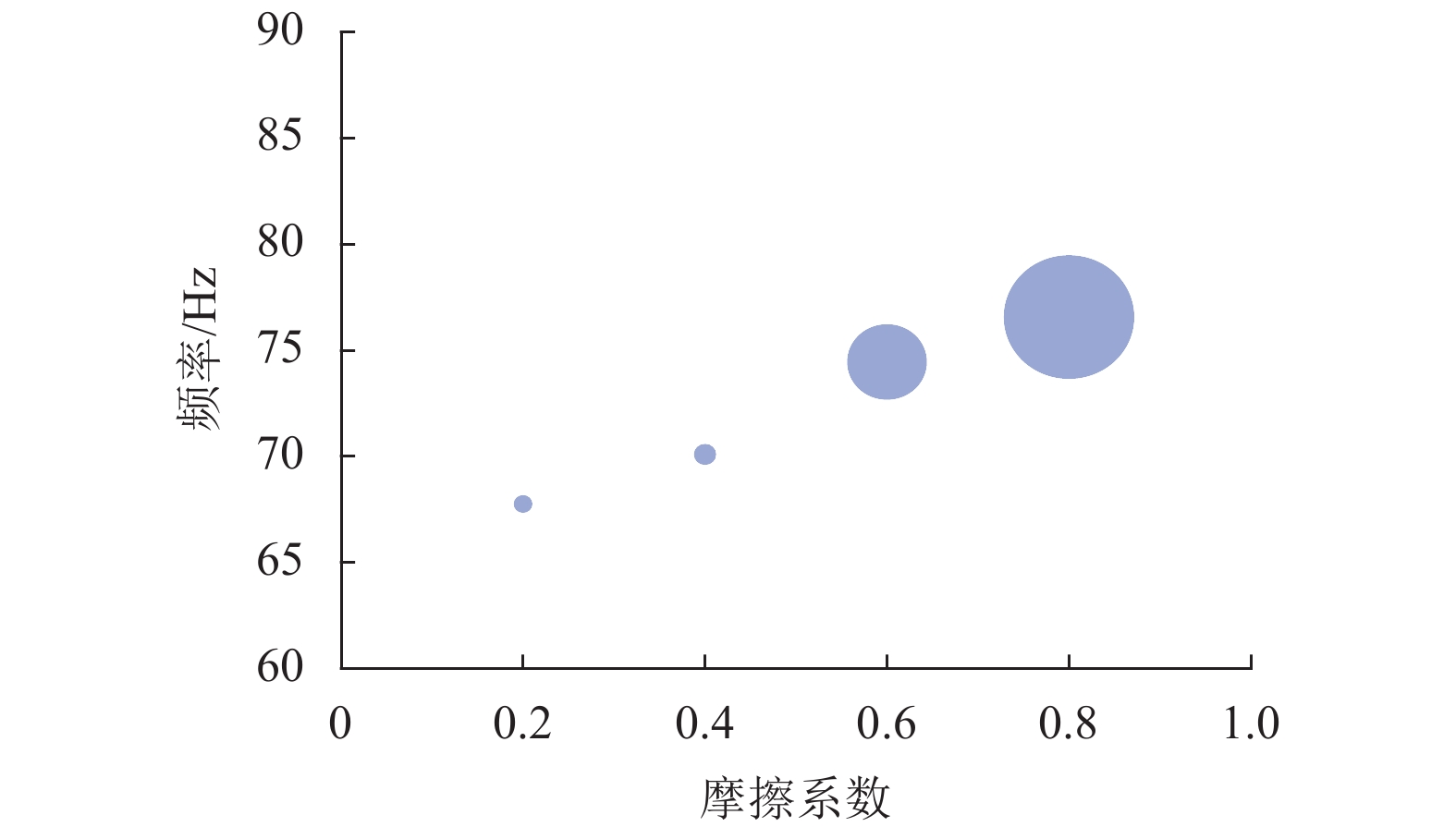
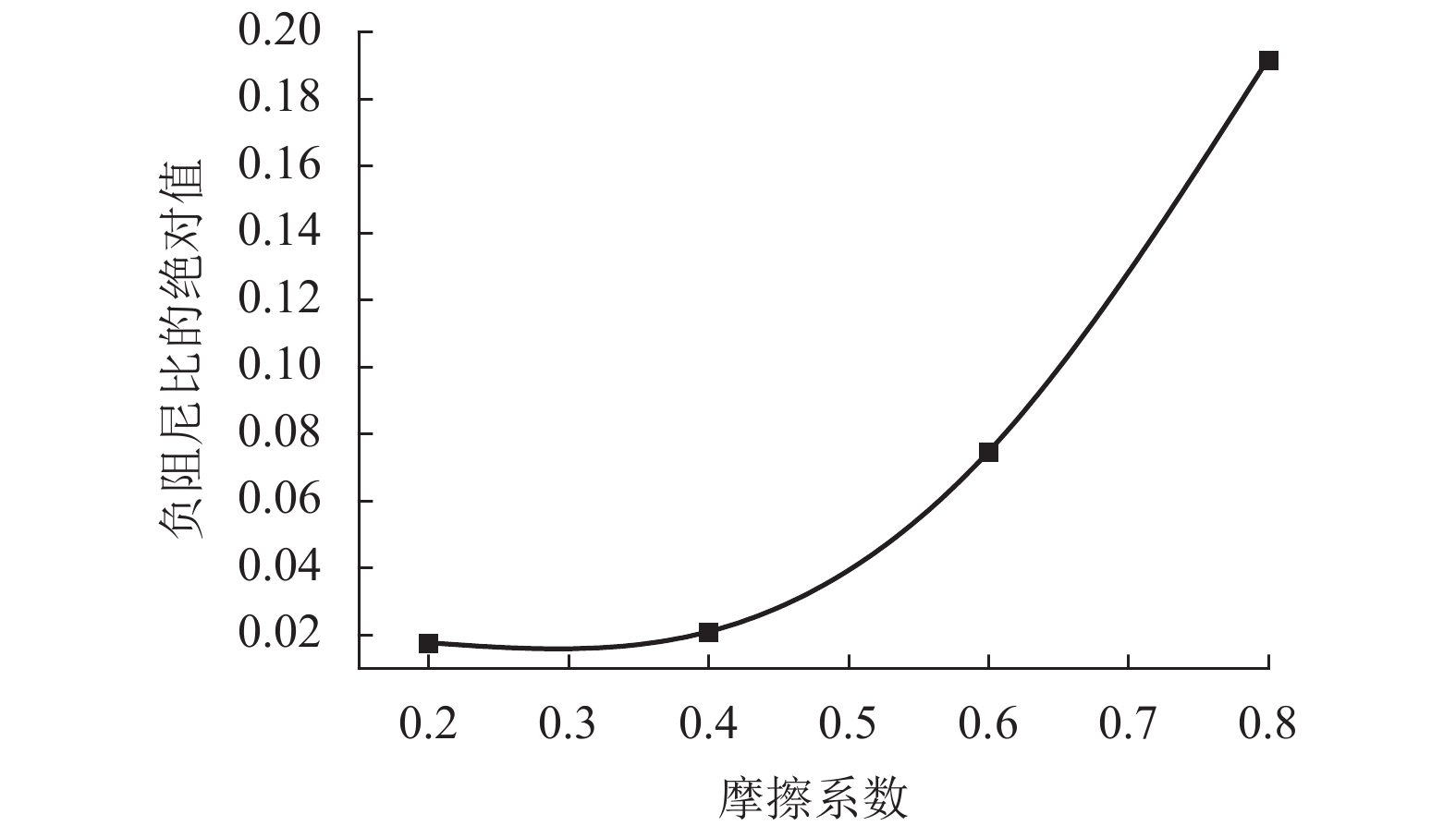
作为轮轨接触摩擦副中最为核心的参数,轮轨表面摩擦系数会随着养护作业状况、干湿情况等因素发生变化. 一般而言,对钢轨表面做涂油处理后,轮轨表面摩擦系数会降至0.2,对钢轨做撒沙处理时,轮轨表面摩擦系数会增加至0.6[9]. 为了更加清晰地揭示摩擦系数对轮轨系统的影响,在本文研究中将摩擦系数扩大至0.8,故此处分析摩擦系数为0.2、0.4、0.6、0.8的4种工况. 进行系统复特征值分析,最小负等效阻尼比计算结果分别为−0.01765、−0.02101、−0.07476、−0.19218,对应的频率分别为68、70、74、77 Hz,如图6所示.
图6中蓝色原点的大小与负等效阻尼比的绝对值正相关,即蓝色圆点越大,对应的轮轨系统更易产生不稳定振动,从而更易导致钢轨波磨发展,如图7所示.
由图7可知:摩擦系数对系统稳定性影响较大,且呈现抛物线式的增长,尤其是系统在轮轨间摩擦系数达到0.4之后,负阻尼比的绝对值急剧增长. 在保证正常轮轨接触和牵引制动需求的前提下,控制轮轨表面摩擦系数在0.4以下,能够较好地避免钢轨波磨的发展. 从神朔重载铁路现场情况来看,长大上坡地段钢轨波磨的产生和发展,与过度撒沙增大轮轨间的摩擦系数有直接关系,导致了系统产生摩擦不稳定自激振动,诱发钢轨波磨病害.
3.3 超高和速度的匹配对轮轨系统不稳定性的影响
根据超高计算方法,当列车运行速度v = 50 km/h,曲线半径R = 400 m时,计算得到超高值为75 mm. 表3列出了5种工况下前3阶负等效阻尼比分布及最小对应的自激振动振型. 附加材料图S7~S11展示了5种工况的详情.
表 3 不同超高下系统最小负等效阻尼比分布及主导振动振型Table 3. Distribution of minimum negative equivalent damping ratio and dominant vibration mode for different superelevation超高 最小负等效阻尼比 主导振型图 欠超高 30 mm −0.01901 
欠超高15 mm −0.00221 
平衡超高 −0.00129 
过超高15 mm −0.00331 
过超高 30 mm −0.02101 
由表3可知:无论是过超高、欠超高还是平衡超高,均出现了不稳定振型;当轨道为过超高状态时,最小负等效阻尼比对应的不稳定振动振型仍出现在内轮-内轨处,当轨道为欠超高状态时,最小负等效阻尼比对应的不稳定振动振型出现在了外轮-外轨处;系统最小负等效阻尼比的绝对值对超高状态更为敏感,超高状态的改变能够很明显地使得系统的稳定性发生变化. 这也很好地解释了部分文献中提到在我国其他一些重载铁路小半径曲线地段发生的钢轨波磨首先出现在曲线外轨上的情况. 上述分析表明,超高状态直接影响波磨的发展,也决定了波磨首先产生在曲线内轨或者外轨上,揭示了钢轨单侧先产生的原因.
综上可知,适当地提高扣件垂横向刚度,控制轮轨摩擦系数在0.4以下以及平衡轨道结构超高能够有效地降低系统发生不稳定振动的可能性,从而抑制波磨的发展.
4. 单侧钢轨波磨的传递与演化分析
通过瞬态动力学数值分析方法模拟单侧钢轨波磨不平顺,研究一侧钢轨波磨产生后对另一侧钢轨的影响. 通过对比分析揭示钢轨波磨的传递与演化规律.
4.1 单侧钢轨波磨不平顺的模拟
根据图1中现场实测钢轨波磨特征进行一般化和抽象化处理,在钢轨内轨表面设置波深为0.5 mm、波长为200 mm的余弦形波磨不平顺,外轨表面则设置为普通平顺状态.
基于重载铁路现场运营的神华号电力机车的实际参数建立瞬态动力学模型,相关参数见附加材料表S4. 由于模型体量较大,车轮与钢轨接触区域网格划分较密,利用Python语言对钢轨表面节点位置进行批量调整,调整后的模型如附加材料图S12所示.
4.2 波磨激发振动的传递
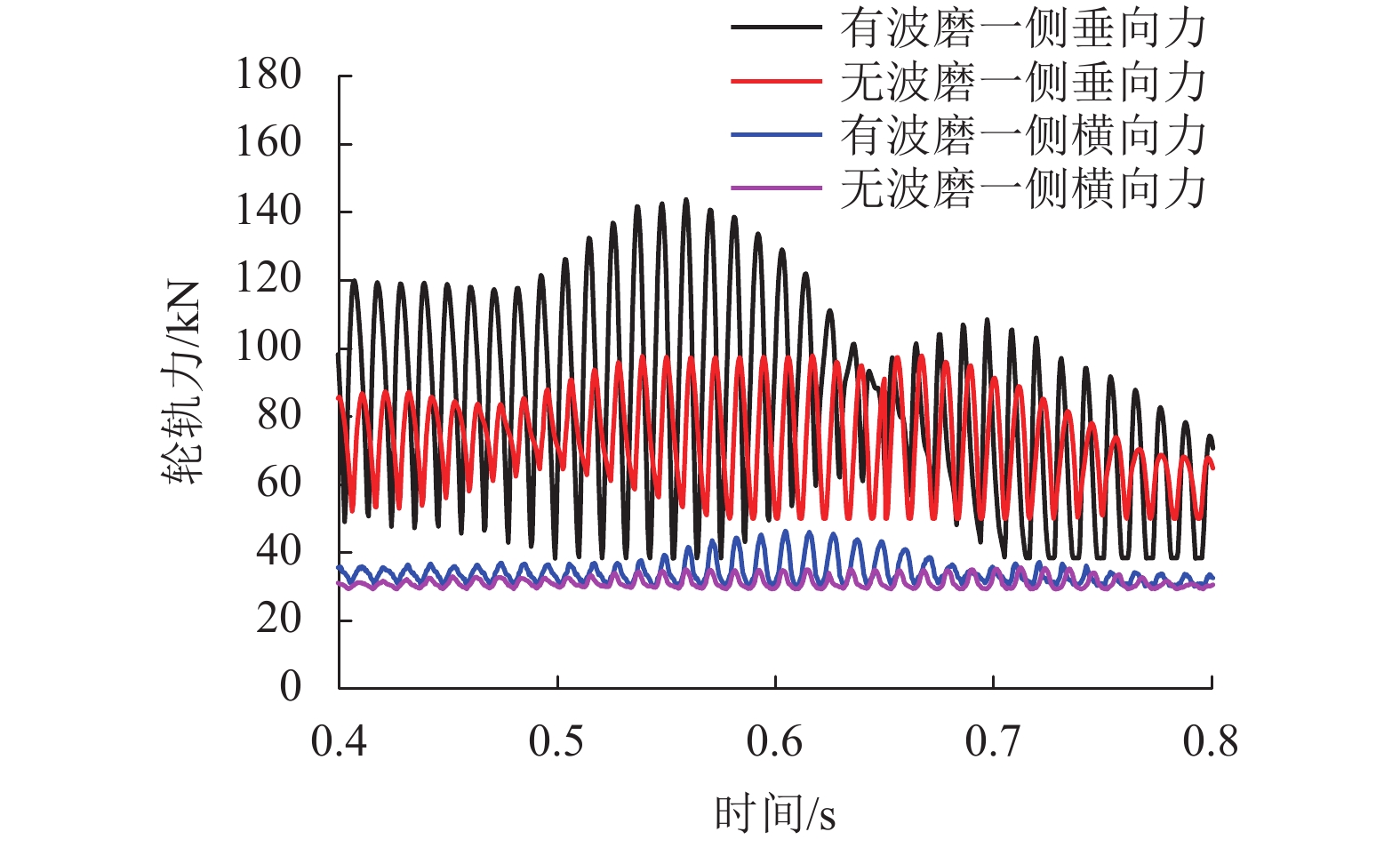
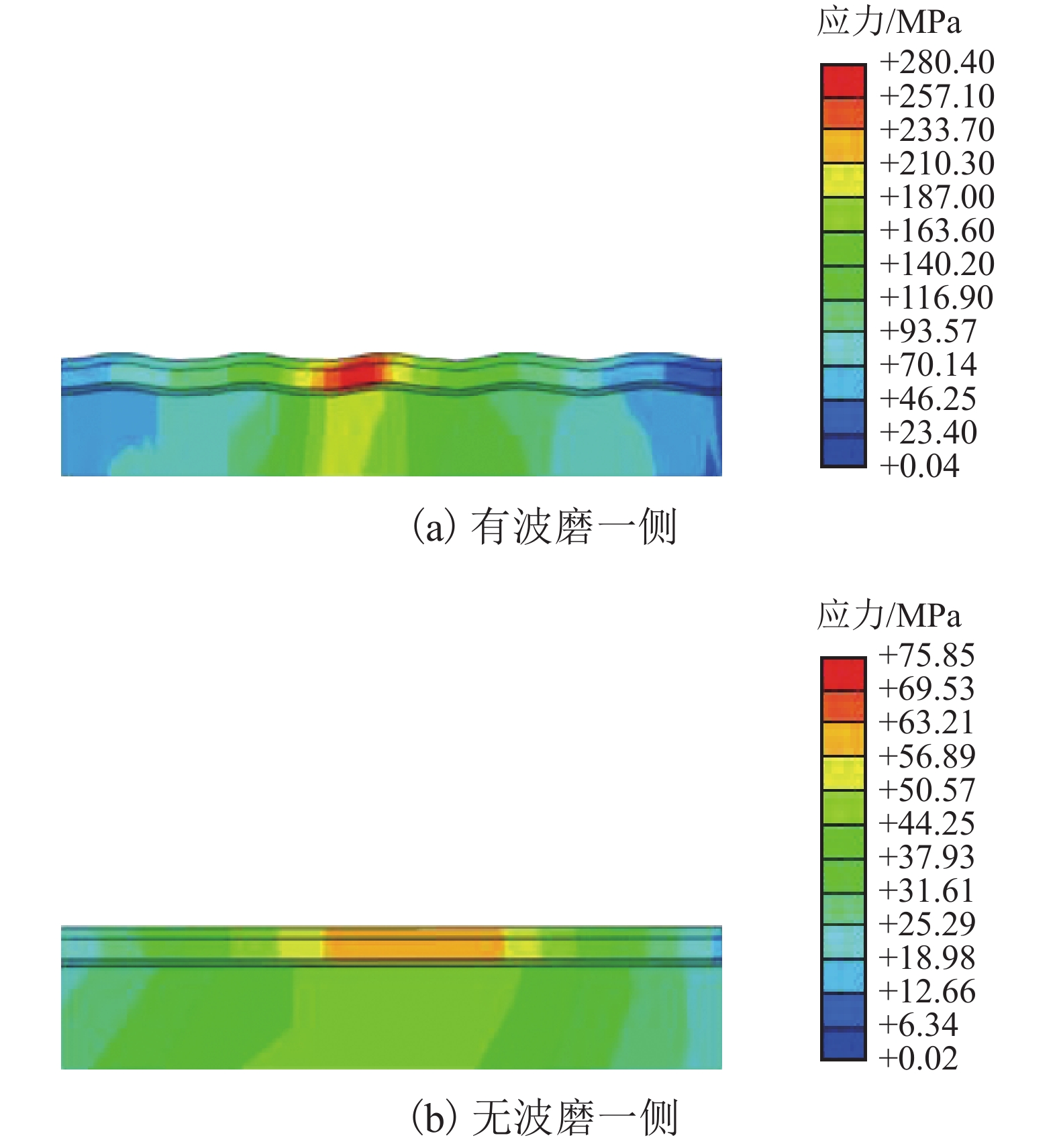
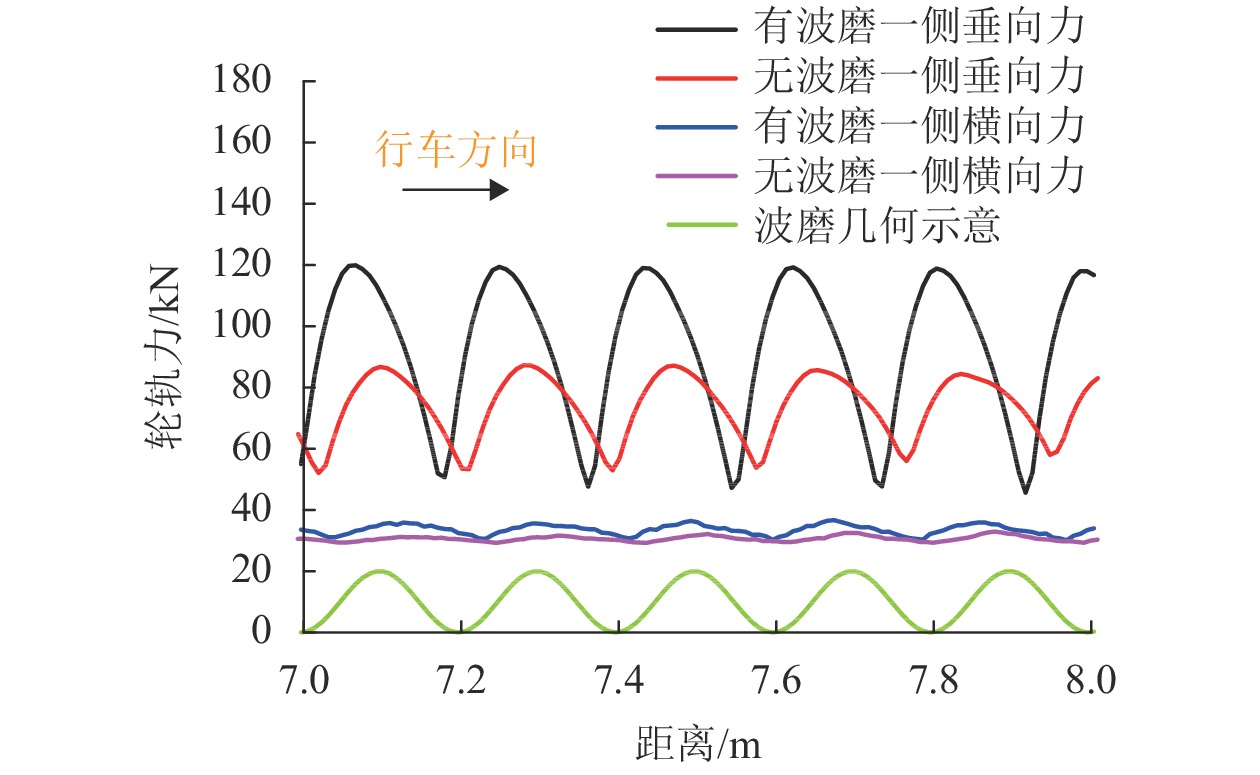
根据波磨在轨道上施加的位置可知,车辆会在0.50~0.65 s经过该波磨段. 图8为车辆以50 km/h的速度通过仅内轨表面有波磨不平顺区段时两侧轮轨垂、横向力瞬态计算结果. 图9为有波磨一侧钢轨和无波磨一侧钢轨应力云图. 由图8、9可以看出:两侧钢轨应力状态相差较大;内轨和外轨的垂、横向轮轨作用力均在波磨的波峰、波谷位置出现了幅值波动,但没有波磨一侧的外轨轮轨作用力波动幅值明显小于有波磨一侧内轨轮轨力,其波动幅值分别为36.2 kN和80.1 kN;轮轨横向作用力因其幅值较小,相应的波动幅值也表现地较小.
观察0.50~0.65 s的波磨施加段还可以发现:在进入波磨地段后,有波磨侧和无波磨侧轮轨作用力的波动变化逐渐表现出了一定的相位差,外侧波动要略晚于内侧的波动,且随着在波磨地段的滚动距离增大相位差也会逐渐变大,因此在通过波磨地段时,无波磨一侧的垂向力整体波动出现相位滞后;同时,横向力波动相较于垂向力波动也发生了一个明显相位差;有波磨一侧的扰动会导致系统发生不稳定振动,从而进一步传递至无波磨一侧;模型中虽然只施加了垂向不平顺,但是横向力也发生了明显的扰动,说明垂向振动的不稳定,也会逐渐传递至横向振动.
为了更清楚地展示系统随波磨状态的振动变化,将时域坐标换算成空间坐标,如图10所示. 由图10可以看出:有波磨一侧的轮轨振动变化和无波磨一侧有明显的相位差,垂向力的振动变化和横向力也有明显的相位差,但变化频率均与波磨几何变化频率相同;轮轨垂横向力的一个变化周期都对应着波磨几何的相应周期. 由此,更好地验证了在小半径曲线地段,钢轨波磨由于不合理的超高设置导致蠕滑力饱和诱发轮轨系统摩擦自激振动,曲线一侧钢轨首先演化出波浪形磨耗,随着轮轨间的振动响应,再逐渐传递至另一侧钢轨.
5. 结 论
1) 首次从钢轨波磨的传递角度揭示波磨演化的全过程,建立的有限元模型能够很好模拟小半径曲线地段轮轨三维接触状态,对轮轨系统进行复特征值计算,分析其不稳定摩擦自激振动状态,从而揭示钢轨波磨先于一侧产生的原因是超高和实际运行速度的不匹配,该结果与实际情况较为吻合.
2) 过超高设置会导致系统在内轮-内轨处发生不稳定摩擦自激振动,欠超高设置则会导致系统在外轮-外轨处发生不稳定自激振动. 该计算结果与我国很多铁路线路钢轨波磨首先产生在单侧钢轨的现象相吻合. 因此,在重载铁路小半径曲线地段设置外轨超高时,应尽量根据该区段车辆实际行驶速度来确定,同时调整摩擦状态,控制摩擦系数在0.4以下. 此外,适当提高扣件刚度,也会减缓钢轨波磨演化和发展.
3) 波磨一侧的扰动会导致轮轨系统发生不稳定振动,并逐渐传递至无波磨一侧,同时垂向不稳定振动也会逐渐传递至横向振动,并进一步放大轮轨动态响应. 该发现进一步揭示了钢轨波磨由一侧钢轨传递至另一侧钢轨的过程,说明不合理的超高设置会导致饱和蠕滑力诱发摩擦自激振动进而产生单侧钢轨波磨,之后向另一侧钢轨传递演化.
备注:附加材料在中国知网本文的详情页中获取.
致谢:神华科技创新项目(SHGF-16-50).
-
表 1 计算结果对比
Table 1. Comparison of calculation results
kN 项目 外轨垂向力 内轨垂向力 现场实测值 126.72 132.11 模型计算值 124.66 128.86 注:现场实测值取多测点平均值,模型计算值取不平顺曲线段平均值. 表 2 扣件刚度计算工况
Table 2. Working conditions of fastener stiffness calculation
MN/m 刚度 工况 1 工况 2 工况 3 工况 4 工况 5 工况 6 工况 7 工况 8 工况 9 横向 40 40 40 60 60 60 80 80 80 垂向 40 80 120 40 80 120 40 80 120 表 3 不同超高下系统最小负等效阻尼比分布及主导振动振型
Table 3. Distribution of minimum negative equivalent damping ratio and dominant vibration mode for different superelevation
超高 最小负等效阻尼比 主导振型图 欠超高 30 mm −0.01901 
欠超高15 mm −0.00221 
平衡超高 −0.00129 
过超高15 mm −0.00331 
过超高 30 mm −0.02101 
-
[1] 温泽峰, 金学松. 钢轨波浪形磨损研究[D]. 成都: 西南交通大学, 2006. [2] 朱波. 大轴重作用下重载铁路路基结构荷载传递规律及设计方法研究[D]. 成都: 西南交通大学, 2018. [3] XIE G, IWNCKI S D. Calculation of wear on a corrugated rail using a three-dimensional contact model[J]. Wear, 2008, 265(9/10): 1238-1248. [4] JIN X, WEN Z, WANG K, et al. Effect of passenger car curving on rail corrugation at a curved track[J]. Wear, 2006, 260(6): 619-633. doi: 10.1016/j.wear.2005.03.016 [5] JIN X, WEN Z. Rail corrugation formation studied with a full-scale test facility and numerical analysis[J]. Proceedings of the Institution of Mechanical Engineers,Part J:Journal of Engineering Tribology, 2007, 221(6): 675-698. doi: 10.1243/13506501JET269 [6] JIN X, WEN Z, WANG K, et al. Effect of a scratch on curved rail on initiation and evolution of rail corrugation[J]. Tribology International, 2004, 37(5): 385-394. doi: 10.1016/j.triboint.2003.07.002 [7] 张厚贵. 北京地铁钢轨波磨的机理及整治方案研究[D]. 北京: 北京交通大学, 2015. [8] CHEN G X, ZHOU Z R, OUYANG H, et al. A finite element study on rail corrugation based on saturated creep force-induced self-excited vibration of a wheelset –track system[J]. Journal of Sound and Vibration, 2010, 329(22): 4643-4655. doi: 10.1016/j.jsv.2010.05.011 [9] 肖祥龙. 基于轮对-钢轨-轨枕系统摩擦自激振动引起的直线轨道钢轨波磨研究[D]. 成都: 西南交通大学, 2012. [10] 刘超,赵鑫,赵小罡,等. 单侧钢轨波磨对两侧轮轨瞬态响应的影响分析[J]. 机械工程学报,2017,53(22): 117-124. doi: 10.3901/JME.2017.22.117LIU Chao, ZHAO Xin, ZHAO Xiaogang, et al. Analyses of transient wheel-rail interactions excited by unilateral rail corrugation[J]. Journal of Mechanical Engineering, 2017, 53(22): 117-124. doi: 10.3901/JME.2017.22.117 [11] 崔晓璐,陈光雄,杨宏光. 轮对结构和扣件刚度对钢轨波磨的影响[J]. 西南交通大学学报,2017,52(1): 112-117. doi: 10.3969/j.issn.0258-2724.2017.01.016CUI Xiaolu, CHEN Guangxiong, YANG Hongguang. Influence of wheelset structure and fastener stiffness on rail corrugation[J]. Journal of Southwest Jiaotong University, 2017, 52(1): 112-117. doi: 10.3969/j.issn.0258-2724.2017.01.016 [12] 中华人民共和国国家铁路局. 轮轨横向力和垂向力地面测试方法: TB/T 2489—2016[S]. 北京: 中国铁道出版社, 2016. [13] SAULOT A, DESCARTES S, DESMYTER D, et al. A tribological characterization of the "damage mechanism" of low rail corrugation on sharp curved track[J]. Wear, 2006, 260(9): 984-995. [14] KURZECK B. Combined friction induced oscillations of wheelset and track during the curving of metros and their influence on corrugation[J]. Wear, 2011, 271(1): 299-310. [15] 李霞. 地铁钢轨波磨形成机理研究[D]. 成都: 西南交通大学, 2012. [16] 王璞,高亮,蔡小培. 重载铁路钢轨磨耗演变过程的数值模拟[J]. 铁道学报,2014,36(10): 70-75. doi: 10.3969/j.issn.1001-8360.2014.10.012WANG Pu, GAO Liang, CAI Xiaopei. Numerical simulation of rail wear evolution of heavy-hual railways[J]. Journal of the China Railway Society, 2014, 36(10): 70-75. doi: 10.3969/j.issn.1001-8360.2014.10.012 [17] 张晴. 重载铁路钢轨磨耗预测研究[D]. 成都: 西南交通大学, 2017. [18] XIAO H, YANG S, WANG H Y, et al. Initiation and development of rail corrugation based on track vibration in metro systems[J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2018, 232(9): 0954409718768956.1-0954409718768956.16. [19] CARLBERGER A, TORSTENSSON P T, NIELSEN J C O, et al. An iterative methodology for the prediction of dynamic vehicle– track interaction and long-term periodic rail wear[J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2018, 232(6): 1718-1730. [20] BROCKLEY C A, KO P L. An investigation of rail corrugation using friction-induced vibration theory[J]. Wear, 1988, 128(1): 99-106. doi: 10.1016/0043-1648(88)90256-6 [21] 中华人民共和国国家铁路局. 铁路轨道设计规范: TB 10082—2017[S]. 北京: 中国铁道出版社, 2017. [22] 刘春阳. 一系悬挂和扣件参数对钢轨波磨影响的仿真分析[D]. 成都: 西南交通大学, 2018. [23] 赵江伟. 摩擦自激振动导致地铁小半径曲线钢轨波磨有限元分析及模型研究[D]. 成都: 西南交通大学, 2018. 期刊类型引用(10)
1. 张峻才,陈光雄,常勇,唐宇,刘崧楠,华浩. 轮轨摩擦自激振动有限元模型的模型优化研究. 润滑与密封. 2025(01): 105-110 .  百度学术
百度学术2. 曾鲁庆,崔大宾,李立. 基于机电液耦合的钢轨打磨模型. 西南交通大学学报. 2025(02): 364-373 .  本站查看
本站查看3. 王平,张洪吉,孙耀亮,安博洋,何庆. 考虑材料温变特性的三维轮轨接触热分析. 西南交通大学学报. 2024(02): 239-246+306 .  本站查看
本站查看4. 何俊华,陈光雄,康熙,李先航,宋启峰,董丙杰. 轮对-轨道系统波磨预测模型的建立及预测分析. 润滑与密封. 2023(01): 79-84 .  百度学术
百度学术5. 郭恭兵,韦凯,谢朝川,肖读杰,王显,张鹏. DZ Ⅲ型扣件铁垫板上横向力传递规律及影响因素分析. 铁道科学与工程学报. 2023(05): 1677-1686 .  百度学术
百度学术6. 崔晓璐,吕东,包鹏羽,李童,漆伟. 山地城市地铁平纵曲线交叠区段钢轨波磨打磨限值研究. 润滑与密封. 2023(06): 1-6+24 .  百度学术
百度学术7. 王罡,叶利宾,陈广生,肖宏. 北京地铁某线路波磨测试及振动测试分析. 工程与建设. 2023(04): 1085-1088+1135 .  百度学术
百度学术8. 折成林. 重载铁路轨底坡设置对轮轨接触及轨面受力的影响. 铁道勘察. 2022(05): 104-109 .  百度学术
百度学术9. 陈光雄. 钢轨波磨预测模型验证工况的研究. 西南交通大学学报. 2022(05): 1017-1023+1054 .  本站查看
本站查看10. 罗易飞,赵鑫,周志军,尹利钧,温泽峰,杨吉忠. 缩尺车轮-环轨试验台轮轨静态接触相似性研究. 中南大学学报(自然科学版). 2022(10): 3901-3911 .  百度学术
百度学术其他类型引用(8)
-






 下载:
下载:

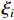


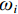

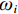
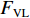
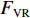
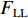
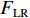
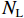
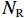
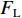
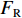
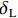
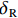
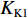
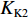

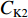
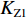
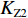
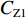
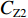








 下载:
下载:




 百度学术
百度学术