Support Stiffness of Magnetic Bearing Based on Unequal Magnetic Circuit Area Design Method
-
摘要:
磁悬浮转子需同时满足抗干扰与共振隔离需求,为了从磁悬浮轴承结构角度奠定设计基础,基于磁悬浮轴承结构参数对支承刚度的影响,对高刚度磁悬浮轴承设计方法展开研究. 首先,通过磁悬浮轴承刚度解析式推导,分析磁悬浮轴承结构参数对支承刚度的影响因素,确定支承刚度优化方向;其次,提出高刚度磁悬浮轴承设计方法,分析支承刚度的优化效果;最后,通过转子固有频率测试及压缩机升频实验,验证所提出方法的可行性. 结果表明:在压缩机样机中,磁悬浮轴承采用不等磁路面积结构,在齿轭宽度比为1.2时,可使轴承在最恶劣工况,即对应最大控制电流时,支承刚度较常规等磁路面积结构提高了25%,压缩机在工况运行区间有效避免共振,为工程应用中磁悬浮轴承刚度优化设计提供参考.
Abstract:The magnetic suspension rotor needs to meet the requirements of anti-interference and resonance isolation at the same time, in order to lay the design foundation from the perspective of magnetic bearing structure, the design method of high stiffness magnetic bearing is studied based on the influence of magnetic bearing structural parameters on support stiffness. Firstly, through the derivation of the analytical formula of the stiffness of the magnetic bearing, the influencing factors of the structural parameters on the support stiffness are analyzed, and the optimization direction of the support stiffness is determined; Secondly, the design method of high stiffness magnetic bearing is proposed, and the optimization effect of support stiffness is analyzed; Finally, the feasibility of the proposed method is verified by rotor natural frequency test and compressor frequency rise experiment. The results show that in the compressor prototype, when the magnetic bearing adopts the structure of unequal magnetic circuit area with the tooth yoke width ratio 1.2, the support stiffness of the magnetic bearing under the worst working condition, that is, the maximum control current, can improve by 25% compared with the equal magnetic circuit area, and the compressor can effectively avoid resonance in the operating range, which provides a reference for the optimization design of the support stiffness of magnetic bearing in engineering application.
-
磁悬浮轴承的支承特性是影响磁悬浮系统稳定性的重要因素. 磁悬浮轴承刚度越高,抗扰动的能力越强[1],为了确保转子高精度悬浮,支承刚度要求最大化设计[2]. 但同时,磁悬浮轴承的支承刚度会影响转子的临界转速与振动模态,刚体模态频率受磁悬浮轴承支承刚度的影响尤为明显[3-6]. 磁悬浮制冷离心压缩机运行频率范围宽,轴承支承刚度设计过高时,易导致转子刚体模态频率与运行频率接近,引起转子共振,因此,支承刚度的设计应同时满足抗干扰性能及刚体模态频率的要求.
目前磁悬浮轴承支承特性影响因素多侧重于分析控制器参数的影响[7-8]. 磁悬浮轴承的结构优化多以承载力为目标,忽略磁饱和、漏磁等影响时,提高承载力的结构参数一般也可以提高支承刚度,但目前多从几何尺寸角度[9-11]或磁饱和角度[12-13]实现承载力优化,此时轴承已工作在非线性区,支承特性反而可能变差,需结合实际特性进行分析. 本文将重点从结构角度进行支承刚度优化研究,提出高刚度磁悬浮轴承设计方法,并通过有限元仿真及实验验证所设计轴承的可行性,为工程应用中的磁悬浮轴承刚度优化设计提供参考.
1. 轴承支承刚度分析
1.1 支承刚度解析
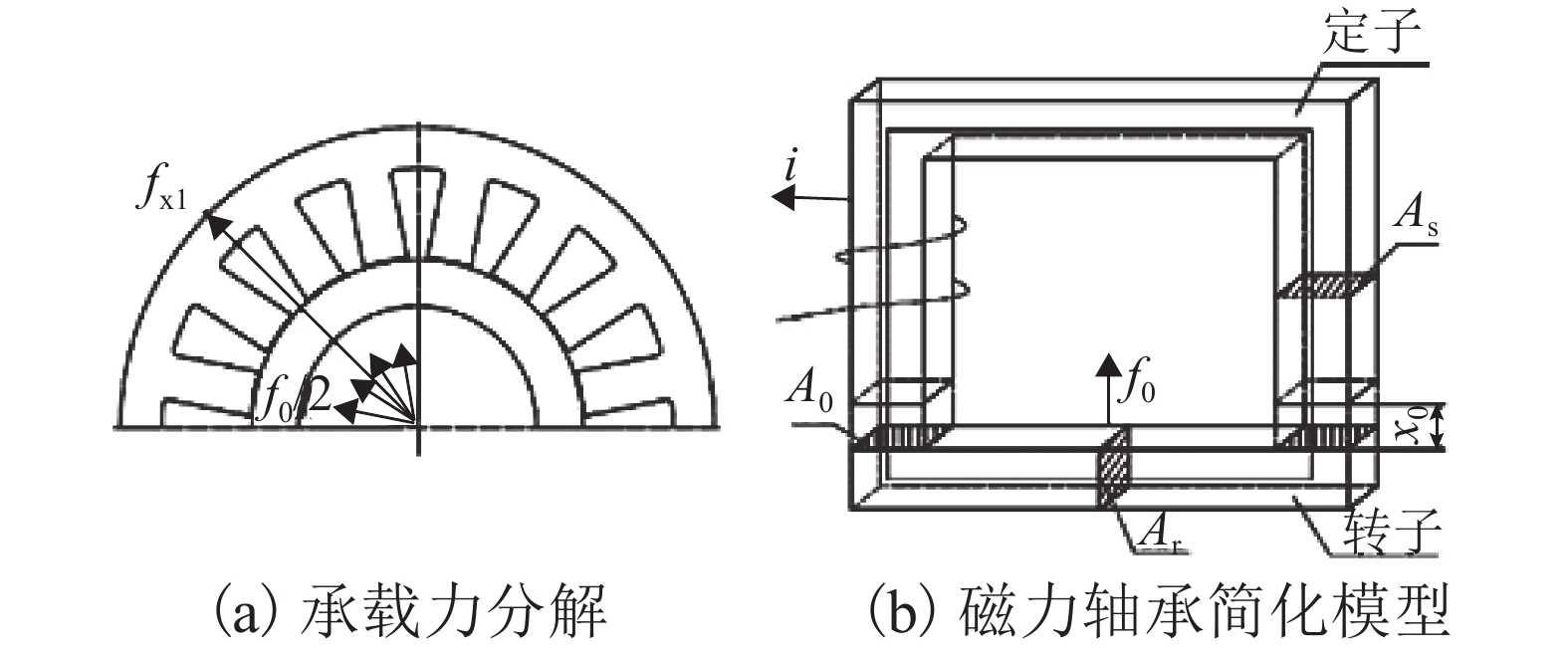
主动式磁悬浮轴承一般有n个齿部(n为磁极数),极性相反的相邻齿部形成磁回路,将轴承分为4相,单相中通入电流,承载力分解如图1(a),每个磁极产生的电磁力合成即可得到单相的承载力,每个磁回路可简化为图1(b)所示模型. 图中:f0为轴承单磁极承载力;fx1为轴承单相承载力;A0为磁路横截面积;As为定子磁路横截面积;Ar为转子磁路横截面积;i为绕组电流;x0为气隙长度.
忽略漏磁、铁心磁阻、磁饱和和材料非线性等,磁力轴承单磁极承载力为
f0=Φ20μ0A0=μ0N2A0i24x20=λ4i2x20, (1) 式中:Φ0c为理想气隙磁通;µ0为空气磁导率;N为绕组匝数;λ为承载力结构系数,
λ =µ0N2A0.制冷压缩机散热效果好,铁心磁密设计更高,此时轴承铁心磁阻对承载力的影响增加,尤其对于大尺寸轴承铁心,虽然铁心中磁导率远大于气隙磁导率,但铁心中磁路平均长度也远大于气隙大小. 根据磁阻计算公式,铁心中磁路平均长度越大、磁导率越小,铁心总磁阻越大,对承载力计算结果的影响也越大.
在忽略漏磁时,可以得到考虑铁心磁阻时的磁通Φ0c,如式(2)所示.
Φ0c=NiRc+R0=Ni(λR+1)R0, (2) {Rc=Rs+R0,Rs=ltμtAt+lyμyAy,Rr=lrμrAr,R0=2x0μ0A0, (3) 式中:Rc为铁心总磁阻;Rs、Rr、R0分别为定子铁心、转子铁心、气隙的磁阻;λR=Rc/R0,为相对磁阻系数;µt、µy、µr分别为定子铁心齿部、轭部和转子铁芯的磁导率;lt、ly、lr分别为定子铁心齿部、轭部和转子铁芯的平均长度;At、Ay分别为定子铁心齿部、轭部的磁路横截面积.
考虑铁心阻抗得到的轴承承载力为
f0c=Φ20cμ0A0=f0(λR+1)2=λff0, (4) 式中:λf为承载力磁阻影响系数.
因此,在铁心磁阻较小时,铁心磁阻对承载力计算影响极小,如λR ≤ 0.01时,λf ≥ 0.98,此时铁心磁阻对承载力计算影响可忽略;但当铁心磁阻较大时,其对承载力的影响不可忽略. 因此,对于尺寸较大或磁密接近饱和的径向主动式磁悬浮轴承,铁心磁阻对承载力会有较大影响,在电磁分析中不可忽略.
根据承载力分解图,考虑铁心磁阻及轴承出力分解,轴承单相承载力fx1如式(5)所示.
{fx1=λfλnf0,λn=n/8∑j=1cos(2j−1)πn, (5) 式中:
λn 为承载力磁极分布影响系数.在差动控制下,轴承磁力为
f=14λλfλn[(i0−ib)2(x0−xb)2−(i0+ib)2(x0+xb)2], (6) 式中:i0为偏置电流;xb为轴承位移;ib为控制电流.
将式(6)线性化为
f=kxxb−kiib, (7) {kx=λλfλni20x30,ki=λλfλni0x20, (8) 式中:kx为轴承的位移刚度;ki为电流刚度.
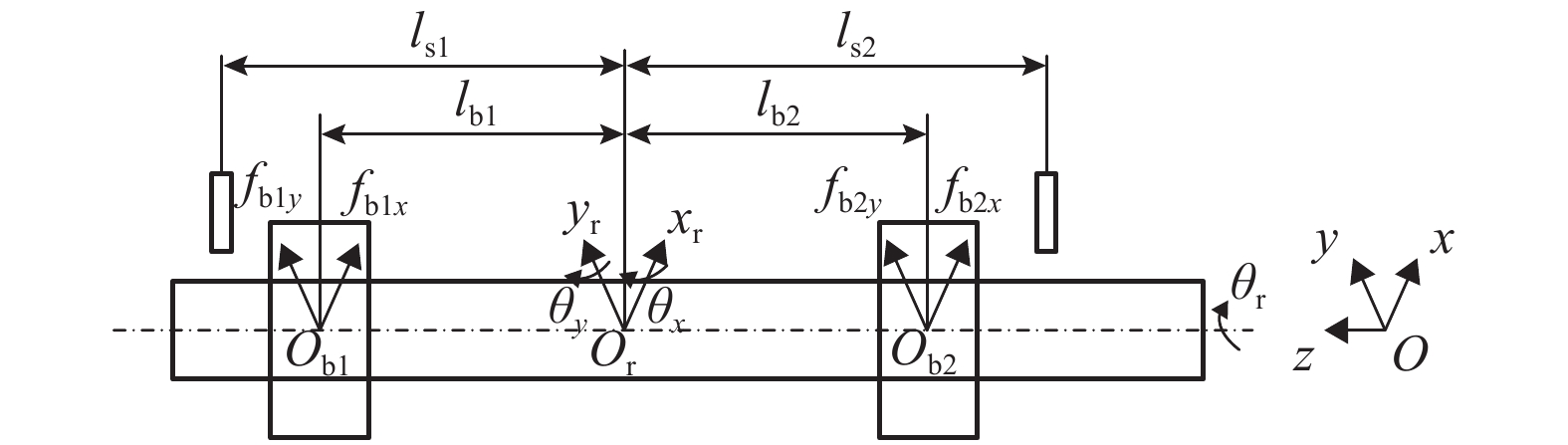
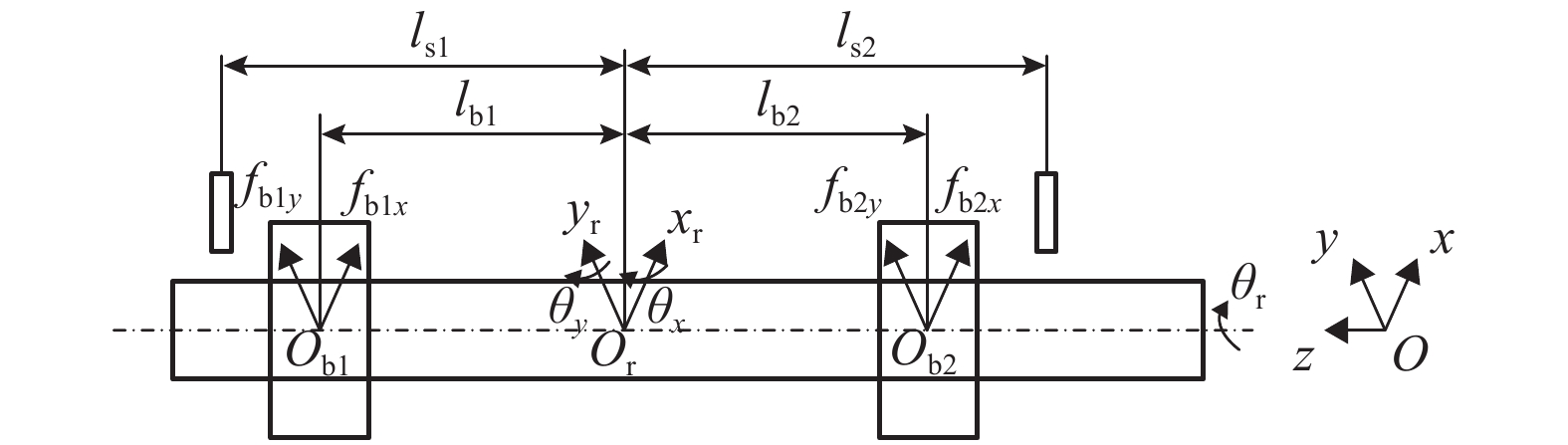
对于磁悬浮刚性转子四自由度系统,以转子质心Or为坐标原点,转子轴线为Oz轴,垂直逆时针45° 方向为Oy轴,水平逆时针45° 方向为Ox轴,建立笛卡尔直角坐标系,如图2所示. 图中:fb1x (fb2x)、fb1y (fb2y)分别为左(右)径向磁悬浮轴承在x、y方向受到的电磁力;lb1、lb2分别为左、右端磁轴承距Or的距离;ls1、ls2分别为左、右端传感器距Or的距离;xr、yr分别为Or在x、y方向的位移;θx、θy、θr分别为转子在yz、xz、xy平面内的转角.
由式(8)可计算得到左(右)径向磁悬浮轴承在x、y方向的位移刚度kxb1x (kxb2x)、kxb1y (kxb2y),左(右)径向磁悬浮轴承在x、y方向的电流刚度kib1x (kib2x)、kib1y (kib2y).
磁悬浮轴承支承承载力矩阵为
fb=kxxb−kiib, (9) 式中:
fb=(fb1x,fb2x,fb1y,fb2y)T ,为承载力矩阵;xb=(xb1,xb2,yb1,yb2)T ,为轴承位移矩阵,xb1 (xb2)、yb1 (yb2)分别为转子在左(右)端磁轴承处x、y方向的位移;ib=(ib1x,ib2x,ib1y,ib2y)T ,为轴承控制电流矩阵,ib1x (ib2x)、ib1y (ib2y)分别为左(右)径向磁悬浮轴承在x、y方向的控制电流;kx = diag(kxb1x, kxb2x, kxb1y, kxb2y),为轴承位移刚度矩阵;ki = diag(kib1x, kib2x, kib1y, kib2y),为轴承电流刚度矩阵.传感器和轴承并不是安装在同一位置,因此,
xb=Kxs, (10) 式中:
xs=(xs1,xs2,ys1,ys2)T ,为传感器检测位移矩阵,xs1 (xs2)、ys1 (ys2)分别为左(右)端传感器检测x、y方向的位移;K为传感器检测位移系数矩阵,如式(11)所示.K=1ls1+ls2[ls2+lb1ls1−lb100ls2−lb2ls1+lb20000ls2+lb1ls1−lb100ls2−lb2ls1+lb2]. (11) 控制器采用PID控制,采用拉普拉斯变换表示为
{Ib(s)=GPID(s)Xs(s),GPID(s)=KP+KIs+KDs, (12) 式中:
Ib(s) 、Xs(s) 分别为ib 、xs 的拉普拉斯变换;GPID(s)为轴承控制器的PID控制函数;KP = diag(KPb1x, KPb2x, KPb1y, KPb2y),为比例系数矩阵,KPb1x (KPb2x)、KPb1y (KPb2y)分别为左(右)径向磁悬浮轴承在x、y方向的比例系数;KI = diag(KIb1x, KIb2x, KIb1y, KIb2y),为积分系数矩阵,KIb1x (KIb2x)、KIb1y (KIb2y)分别为左(右)径向磁悬浮轴承处x、y方向的积分系数;KD= diag(KDb1x, KDb2x, KDb1y, KDb2y),为微分系数矩阵,KDb1x (KDb2x)、KDb1y (KDb2y)分别为左(右)径向磁悬浮轴承在x、y方向的微分系数. 式(9)的复频域形式为
Fb(s)=kxXb−ki(KP+KIs+KDs)K−1Xb(s), (13) 式中:
Fb (s)、Xb (s)分别为fb 、xb 的拉普拉斯变换.将磁悬浮轴承出力等效为弹性支承系统,则磁悬浮轴承出力可表示为
fb=−kbxb−cb˙xb, (14) 式中:
kb 为等效支承刚度;cb 为等效支承阻尼.式(13)转换为时域形式后,与式(14)对比得到
{kb=kiKPK−1−kx,cb=ki(KD−KIω2)K−1, (15) 式中:
ω 为频率.因此,转子结构一定时,轴承的等效支承刚度由轴承结构、偏置电流和控制参数共同确定.
1.2 影响因素分析
对于制冷压缩机,支承刚度需计入轴承铁心磁阻的影响. 根据式(15),以左侧径向轴承x方向为例,支承刚度为
kb1xx=λλfλnx20b1(ls1+lb2lb1+lb2KPb1xi0b1x−i20b1xx0b1), (16) 式中:i0b1x为左轴承x方向的偏置电流;x01为左轴承x方向的气隙.
对于磁悬浮制冷压缩机,出于客户应用需求及成本考虑,在满足性能要求的情况下,要求尽可能减小压缩机体积,因此,磁悬浮轴承结构设计中也有较严格的体积限制. 可以看出,从结构角度,在轴承定转子外径、厚度一定的条件下,支承刚度主要受气隙、绕组匝数、磁极面积及磁极数目的影响. 下面分别分析各结构参数对支承刚度的影响.
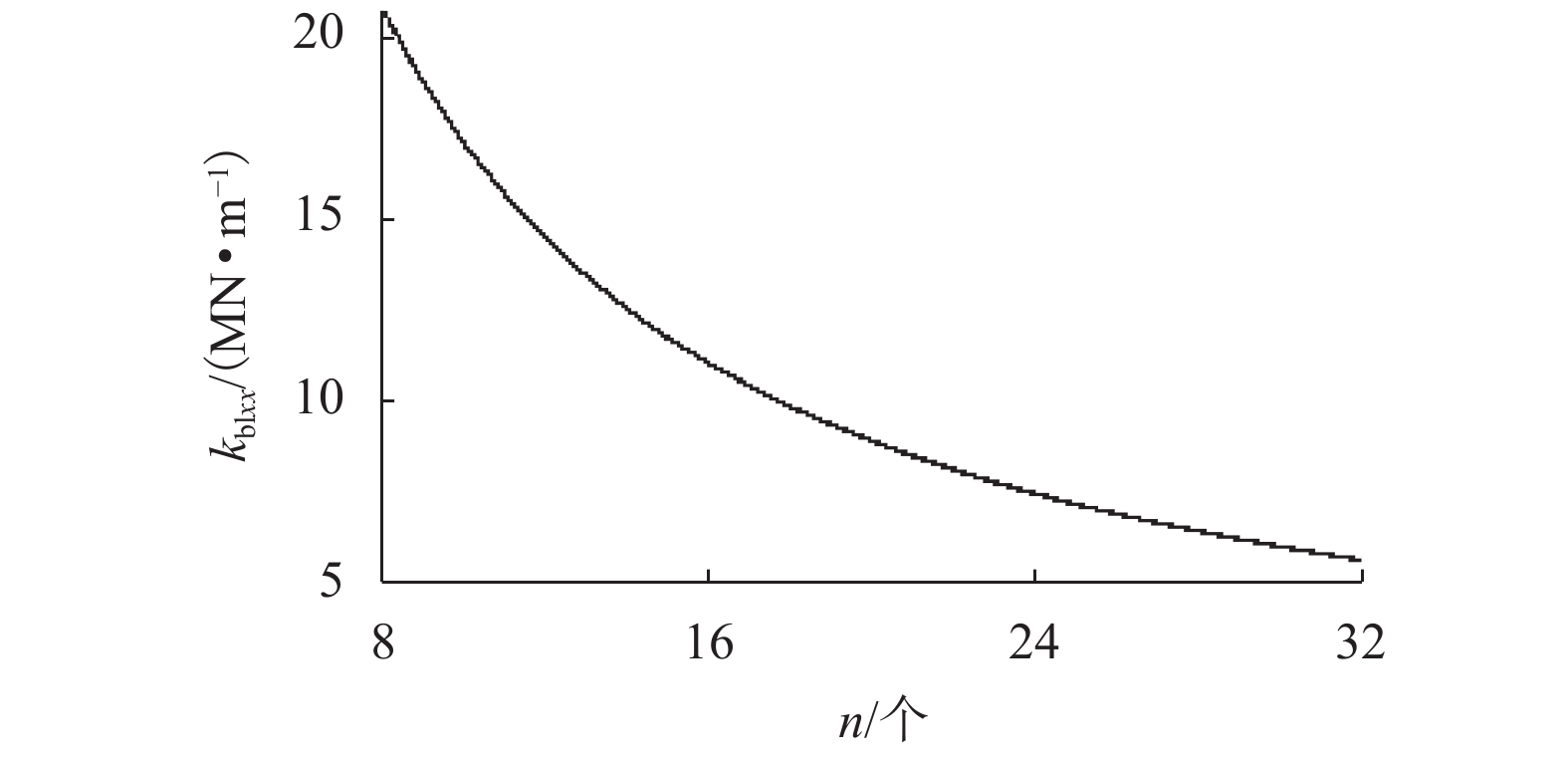
1) 磁极数
在磁极分布不同时,保证总的磁极面积Atotal一定,则满足
λλn=μ0N2Atotalnn/8∑j=1cos(2j−1)πn. (17) 支承刚度随磁极数变化的关系曲线如图3所示.
磁极数n越小,λ与λn乘积越大,则支承刚度越大. 由于磁极数目一般为4的整数倍,而4极结构在两个径向方向的耦合严重,因此,在满足其他条件的情况下,优选8极结构.
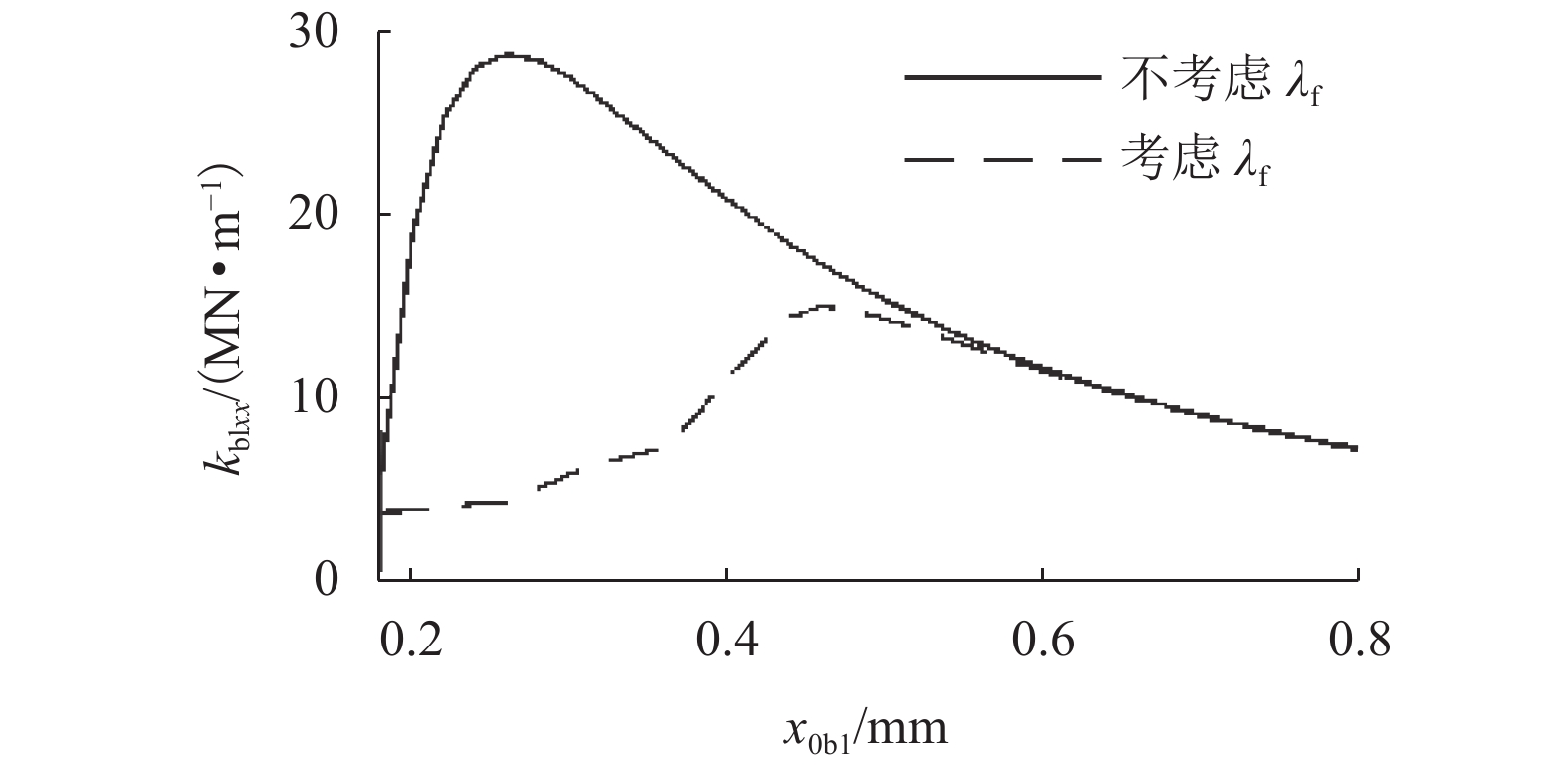
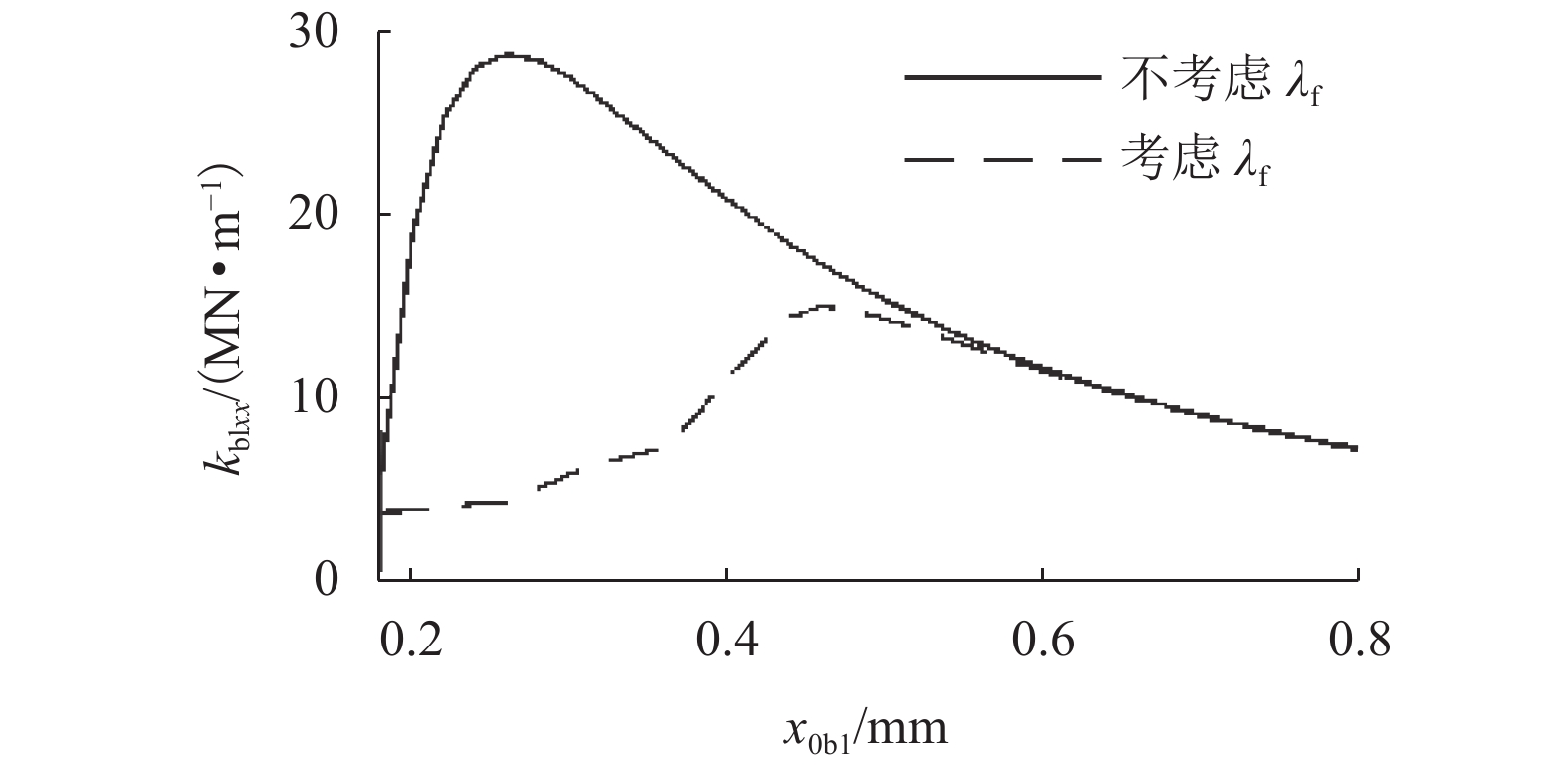
2) 气隙
支承刚度随气隙变化的关系曲线如图4所示.
由图4可以看出,支承刚度随气隙先增大后减小.
不考虑铁心磁阻的影响,即λf = 1,当
∂kb1xx∂x0b1=0 ,即x0b1=3i0b1x(lb1+lb2)2KPb1x(ls1+lb2) 时,支承刚度值最大,此时kb1xx=4λλfλnK3Pb1x(ls1+lb2)327i0b1x(lb1+lb2)3 . 由于支承刚度值最大所对应气隙值较小,因此可认为需减小气隙以提高支承刚度.而考虑磁阻影响,即计入λf后,减小气隙时,定子齿部、定子轭部、转子铁心磁阻增加,气隙磁阻减小,λf减小,支承刚度反而可能减小. 同时,受限于加工、装配可行性及运行可靠性等因素,气隙不宜设计过小,因此,从气隙角度进行轴承刚度优化设计效果不佳.
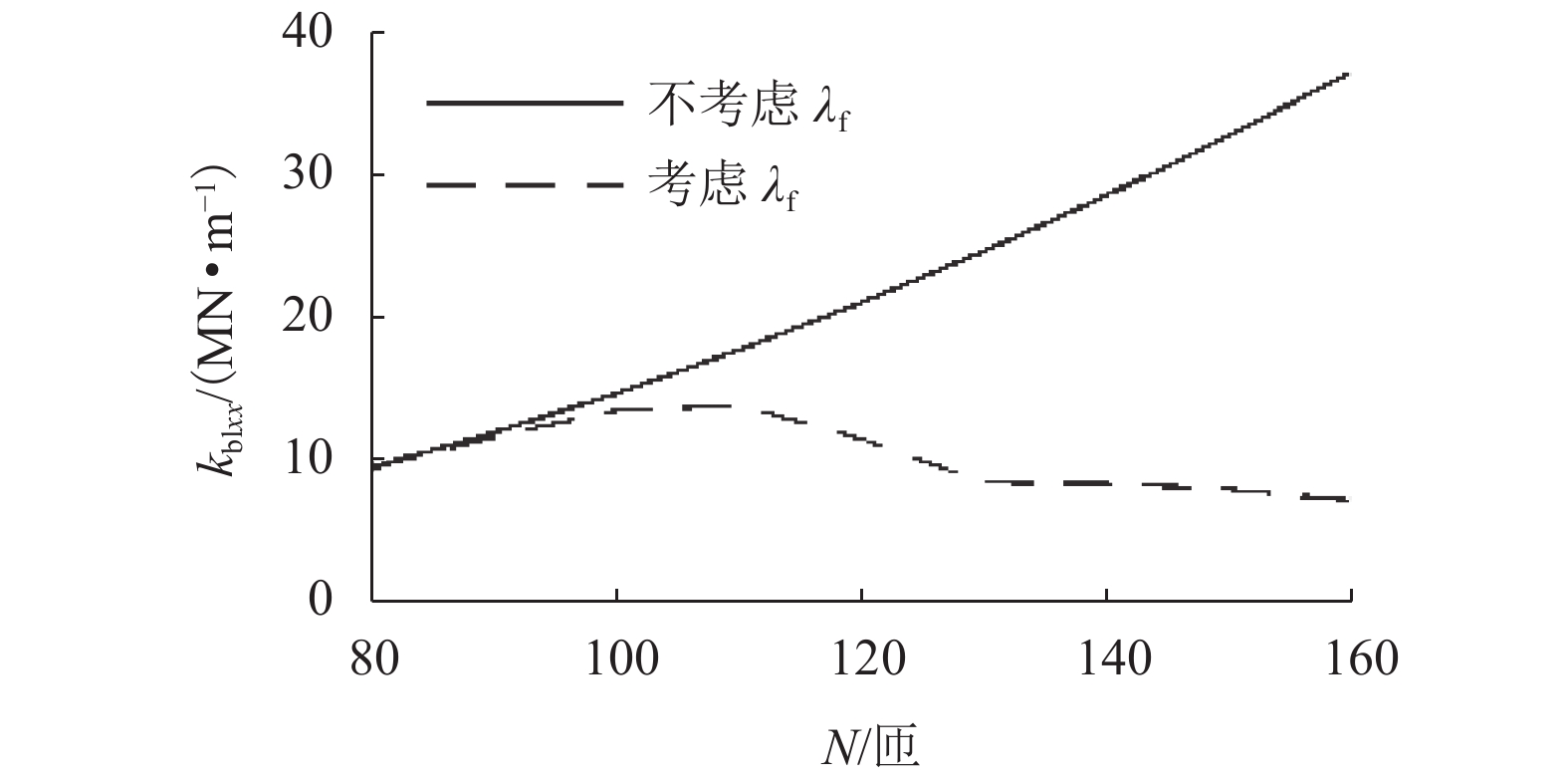
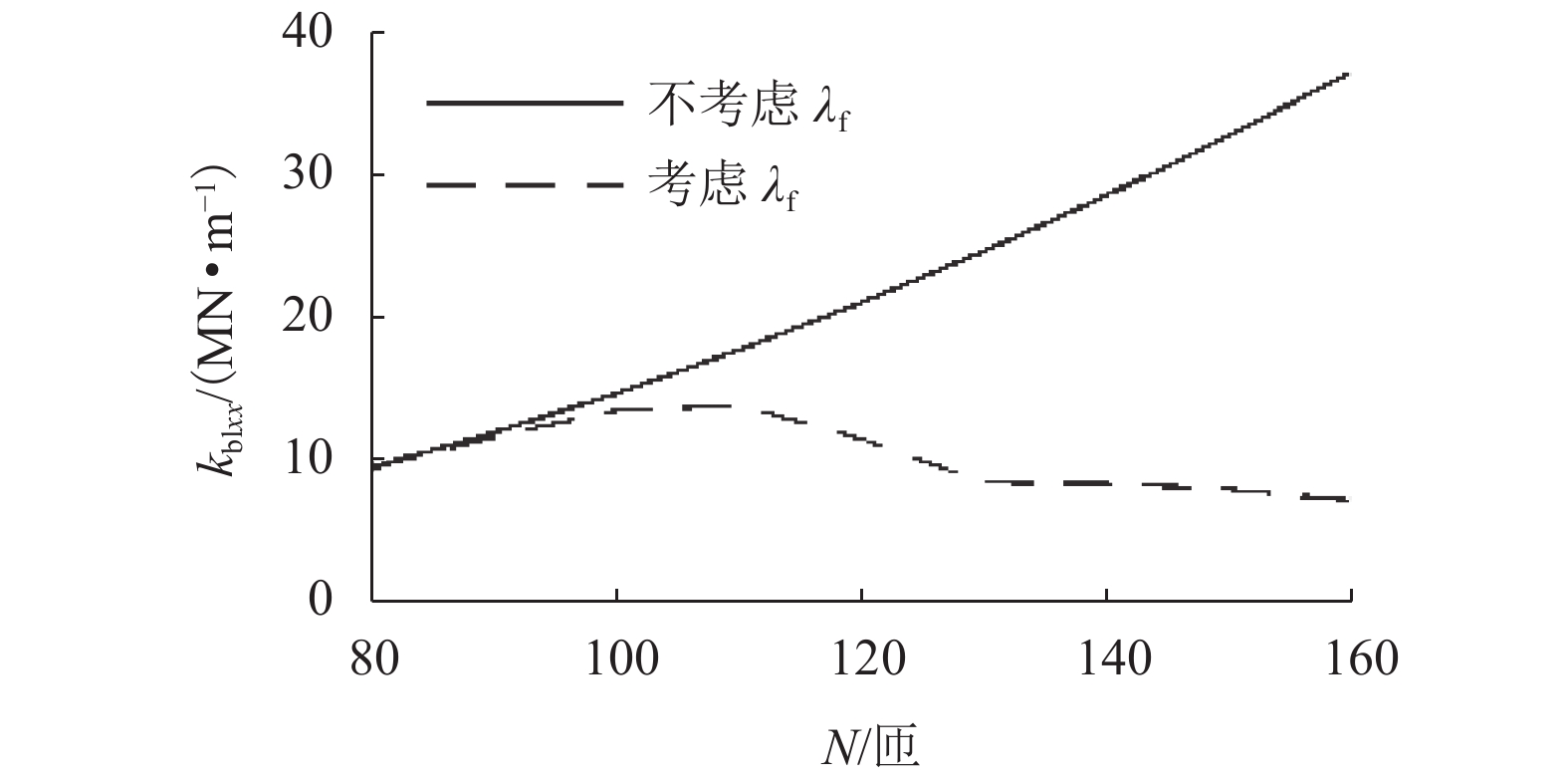
3) 绕组匝数
支承刚度随绕组匝数变化的关系曲线如图5所示.
不考虑铁心磁阻的影响,即λf = 1时,支承刚度与绕组匝数的平方成正比关系,当N > 0时,支承刚度随着绕组匝数N增大而增大.
而考虑磁阻影响,即计入λf后,铁心结构尺寸一定时,增加绕组匝数会导致定子齿部、定子轭部、转子铁心磁阻增加,气隙磁阻不变,λf减小,支承刚度先增加后减小. 同时,增加绕组匝数会导致槽满率提高,提高制作难度,因此,从绕组匝数角度进行轴承刚度优化设计效果同样不佳.
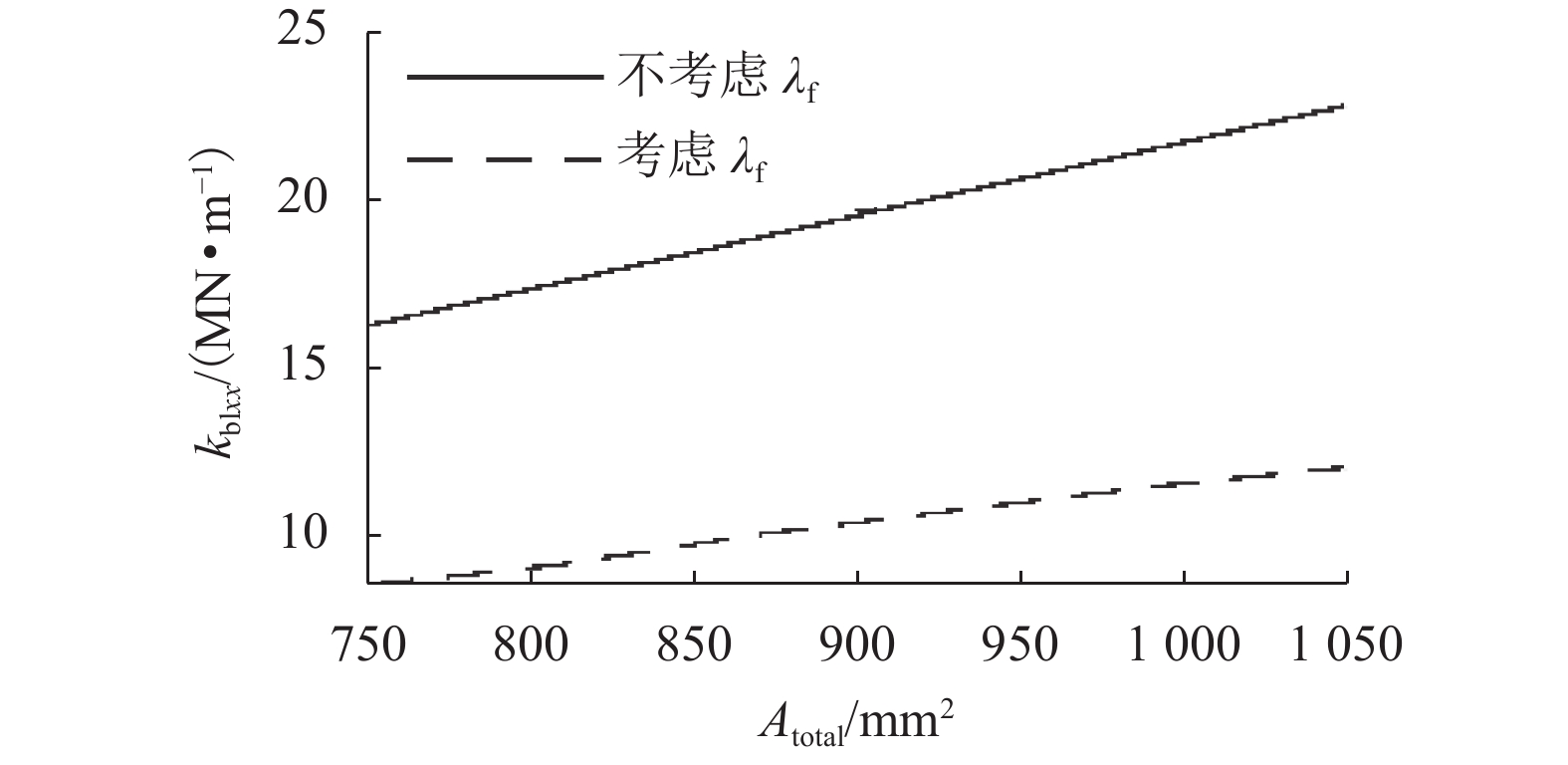
4) 磁极面积
支承刚度随磁极面积变化的关系曲线如图6所示.
支承刚度与磁极面积成正比关系,支承刚度随着磁极面积增大而增大. 一定范围内增大磁极面积对铁心磁阻的影响较小,可有效提高轴承支承刚度.
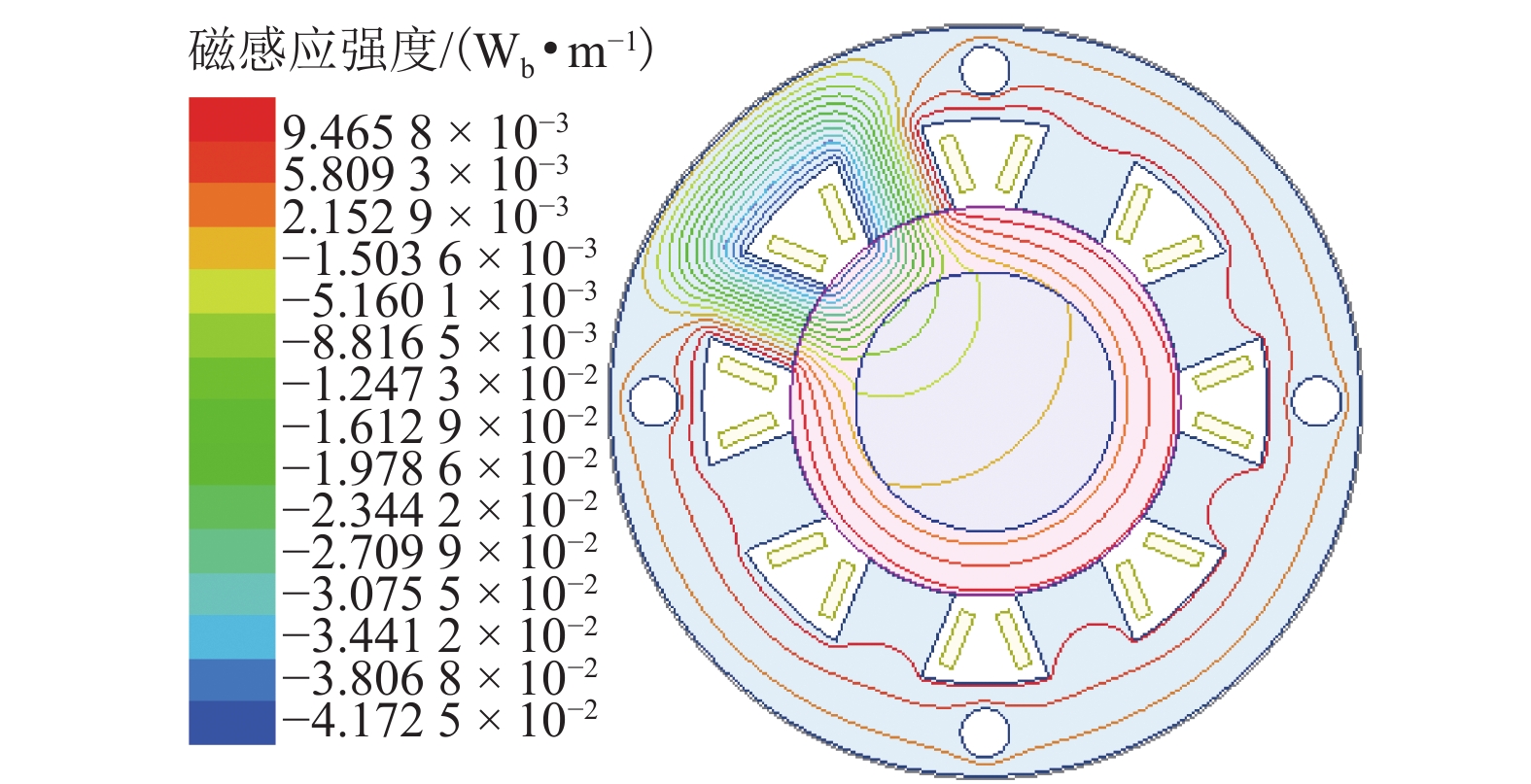
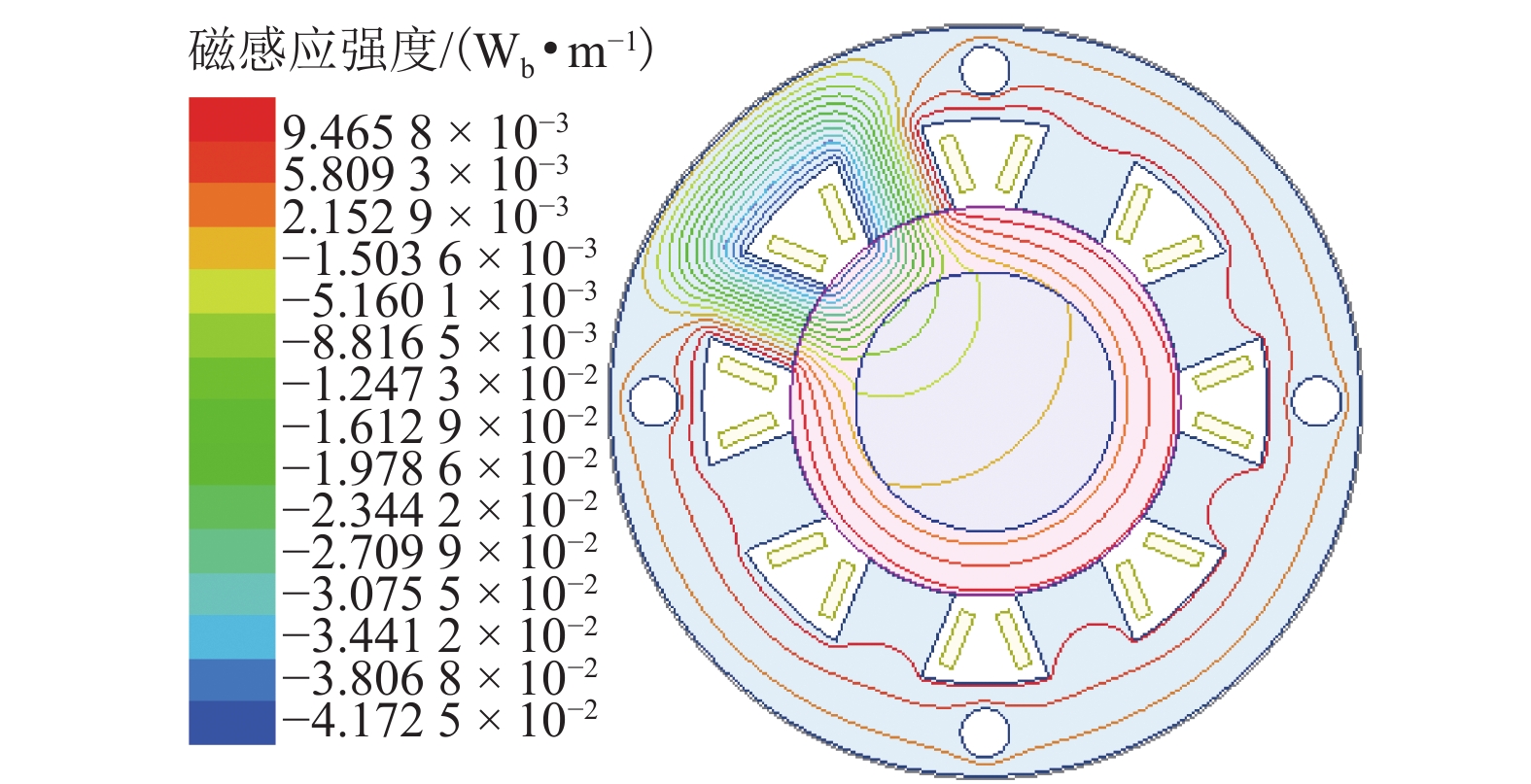
常规磁悬浮轴承设计时,一般取齿部、轭部面积相等,此时增大磁极面积会导致线圈腔面积减小,相同绕组匝数的情况下槽满率明显提高,难以满足制作工艺需求. 同时,对于整体式轴承,定子磁极间存在漏磁,其磁力线分布如图7所示. 齿部、轭部面积相等时, 齿部磁密高于轭部磁密,使得轭部材料利用率不高.
因此,可采用不等磁路面积结构,即增加齿部面积的同时减小轭部面积,通过齿轭宽度的合理设计,保证了线圈腔面积和槽满率不变,定子齿部磁阻减小,轭部磁阻增加,转子和气隙磁阻不变,λf基本不变,达到轴承整体磁饱和程度不变的同时提高支承刚度的效果.
由上述分析可知:在轴承外形尺寸不变的情况下,从轴承结构设计角度,可通过减小气隙大小、增加绕组匝数、增大磁极面积(齿部面积)、减少磁极数目来提高轴承的支承刚度. 但考虑磁阻影响及工艺性要求,针对磁悬浮制冷压缩机,磁悬浮轴承优选8极结构,且在外形尺寸一定的条件下,考虑采用不等磁路面积结构优化设计磁轴承支承刚度.
2. 支承刚度设计方法研究
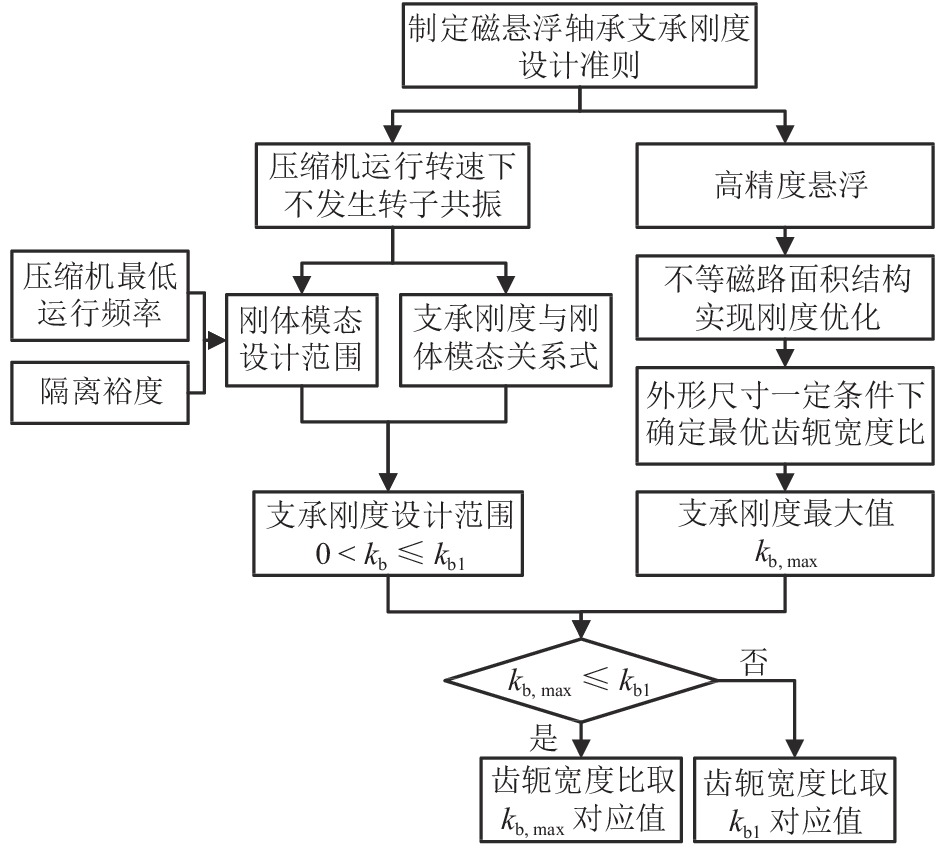
基于上述分析,从不等磁路面积结构角度进行支承刚度优化设计研究,提出一种针对于制冷离心压缩机的高刚度磁悬浮轴承设计方法,结合制冷离心压缩机的特点,明确支承刚度设计准则为:确保离心压缩机工况运行时转子不发生共振,且能高精度悬浮(支承刚度尽可能大),其设计流程如图8所示. 图中:kb、kb1分别为轴承设计支承刚度和满足刚体模态频率隔离裕度极限值时对应的支承刚度. 首先,基于压缩机运行频率和隔离裕度要求,确定支承刚度设计范围;然后,考虑铁心磁阻影响,优选从磁极面积角度设计成不等磁路面积结构实现支承刚度优化;最后,比较支承刚度设计范围和优化最大值,确定齿轭宽度比最优尺寸参数.
2.1 支承刚度设计范围确定
磁悬浮刚性转子四自由度系统运动方程为
M¨xr+C˙xr+Afb+fd=0, (18) 式中:转子质心位移矩阵xr、质量矩阵M、陀螺矩阵C、承载力系数矩阵A、干扰力矩阵fd分别为
xr=(xr,yr,θx,θy)T,fd=(−fx,−fy,−Mx,−My)T, M=[m0000m0000Jd0000Jd],C=[00000000000ωrJp00−ωrJp0], A=[−1−10000−1−100lb1−lb2−lb1lb200], 其中:m为转子质量;Jd为绕质量中心的截面惯性矩;Jp为绕质量中心的极惯性矩;ωr为转子的旋转角速度;fx、fy为作用在质心处向x、y方向的外扰力(重力、不平衡力、冲击力等);Mx、My分别为相对于质心处绕x、y轴的外扰力矩.
根据图2轴承转子与质心位置的几何关系,可以得到
xr=Bxb, (19) 式中: B为磁轴承位移系数矩阵,如式(20)所示.
B=1lb1+lb2[lb2lb10000lb2lb100−111−100]. (20) 将式(14)、(19)代入式(18)得到
M¨xr+(C−AcbB−1)˙xr−AkbB−1xr+Fd=0. (21) 由式(21)可得到固有频率矩阵
ωn 满足ω2n=−M−1AkbB−1. (22) 为保证转子在工作转速下能够稳定运行,要求转子的固有频率与工作转速之间保留一定的隔离裕度,对于刚性转子应满足
ωni⩽ (23) 式中:ωni为刚体模态频率;Si为刚体模态频率的隔离裕度.
因此,对应得到支承刚度设计应满足
0<k_{\rm{b}} \leqslant k_{\rm{b} 1} \text{.} (24) kb1对应刚体模态频率极限值为
\dfrac{{{\omega _{\min }}}}{{1 + {S_{\text{i}}}}} ,ωmin为最低运行转速.对于左(右)轴承x方向的支撑刚度kb1xx、kb2xx与左(右)轴承y方向的支承刚度kb1yy、kb2yy,当kb1xx =kb2xx, kb1yy = kb2yy时,
{k_{{\text{b}}1}} = \min \left\{ {\frac{m}{2}{{\left( {\frac{{{\omega _{\min }}}}{{1 + {S_{\text{i}}}}}} \right)}^2},\frac{{{J_{\text{d}}}}}{{l_{{\text{b}}1}^2 + l_{{\text{b}}2}^2}}{{\left( {\frac{{{\omega _{\min }}}}{{1 + {S_{\text{i}}}}}} \right)}^2}} \right\} . (25) 2.2 不等磁路面积轴承设计
基于前述分析,将采用不等磁路面积结构来优化支承刚度. 结构参数的改变会影响到λF,当齿轭宽度比过大时,轭部尺寸很小,极易造成饱和,即λF明显减小,此时支承刚度反而可能减小,因此,要在磁悬浮轴承工作范围内综合考虑磁饱和及磁极面积的影响,确定最优齿轭宽度比.
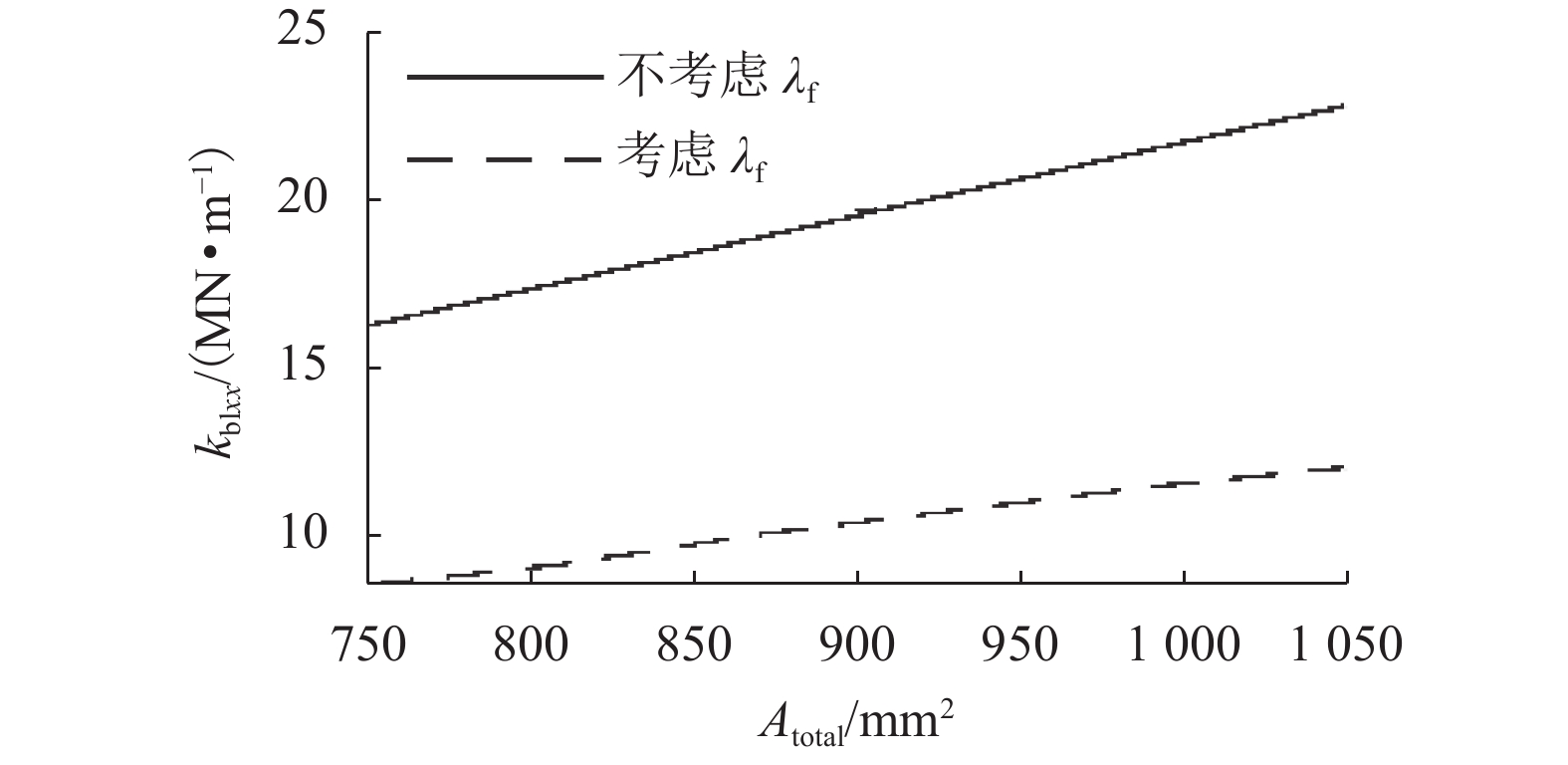
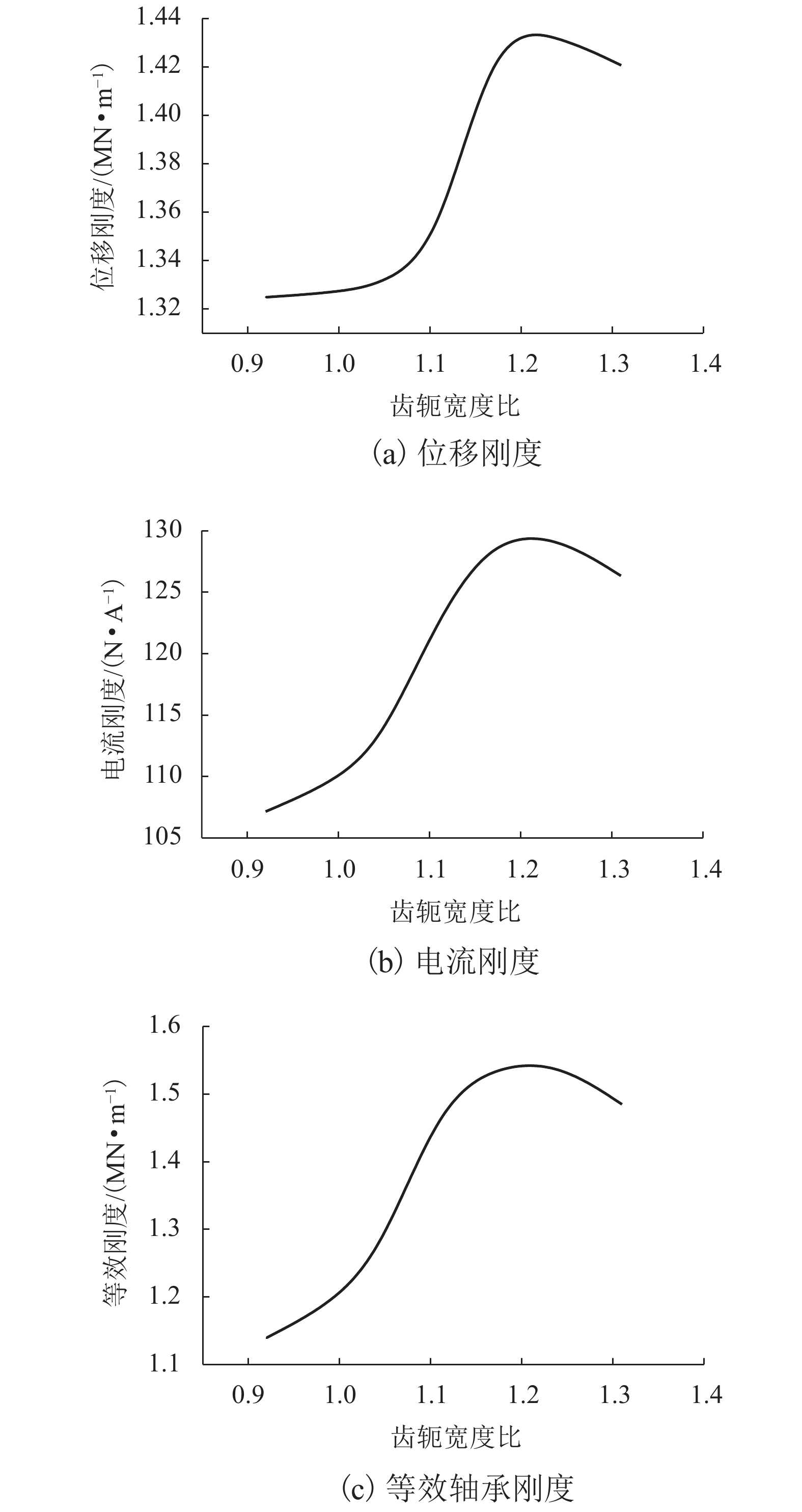
为探究磁极宽度对电磁特性的影响,以应用于150 冷吨磁悬浮离心压缩机的磁悬浮轴承为例, 在相同线圈槽面积时,利用有限元分析,仿真比较齿轭宽度比对轴承电磁性能的影响.
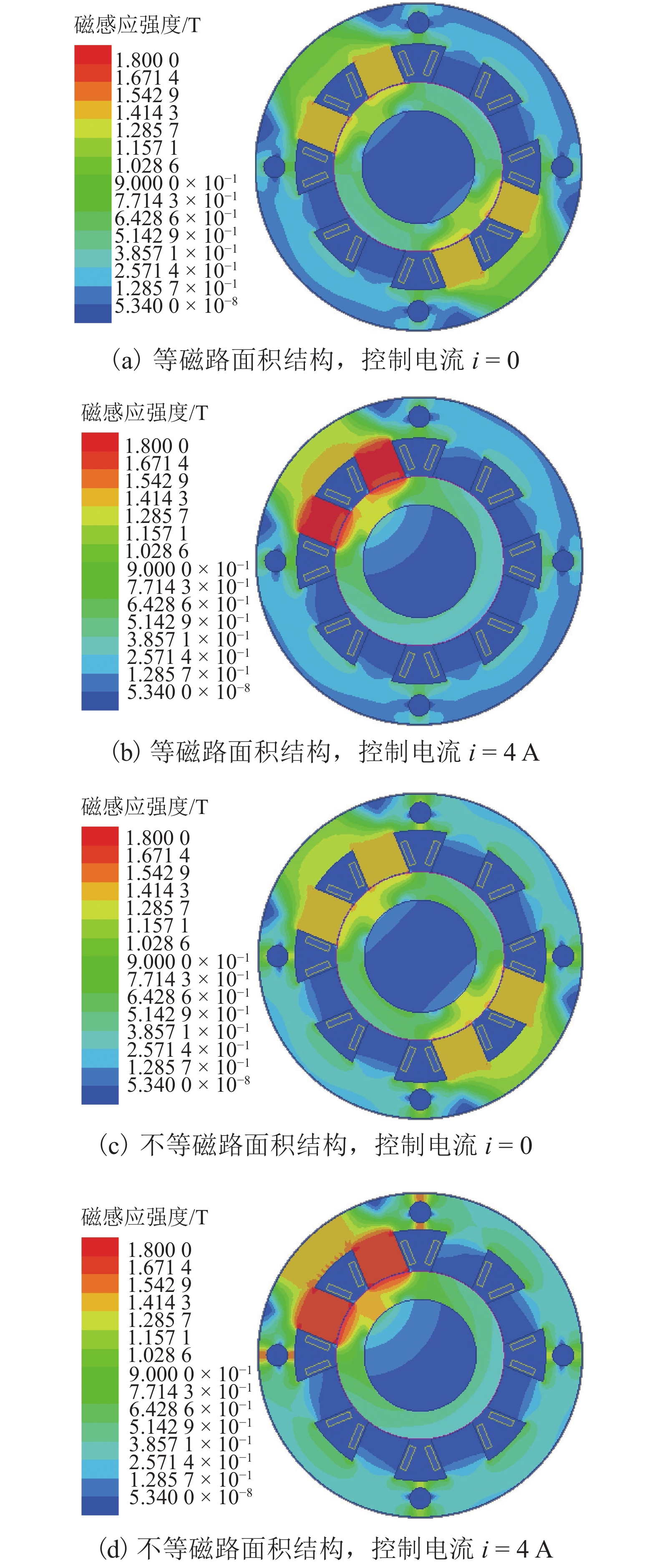
该轴承所支承转子功率为100 kW,额定转速为500 Hz,最低运行转速为325 Hz,根据式(25)计算得到kb1=2.2 × 107 N/m,通过有限元仿真,磁悬浮轴承采用差动控制方式,在不同控制电流下的磁密云图如图9所示.
由图9可以看出:控制电流增大使得轴承出力方向的磁极磁密增大,饱和程度加深. 采用不等磁路面积结构,可明显减小齿部磁密,同时轭部磁密略有增大但仍未达到饱和.
在计算轴承等效刚度时,控制系统的比例系数Kp = 20000,电流刚度ki和位移刚度kx取电磁仿真的实际值.
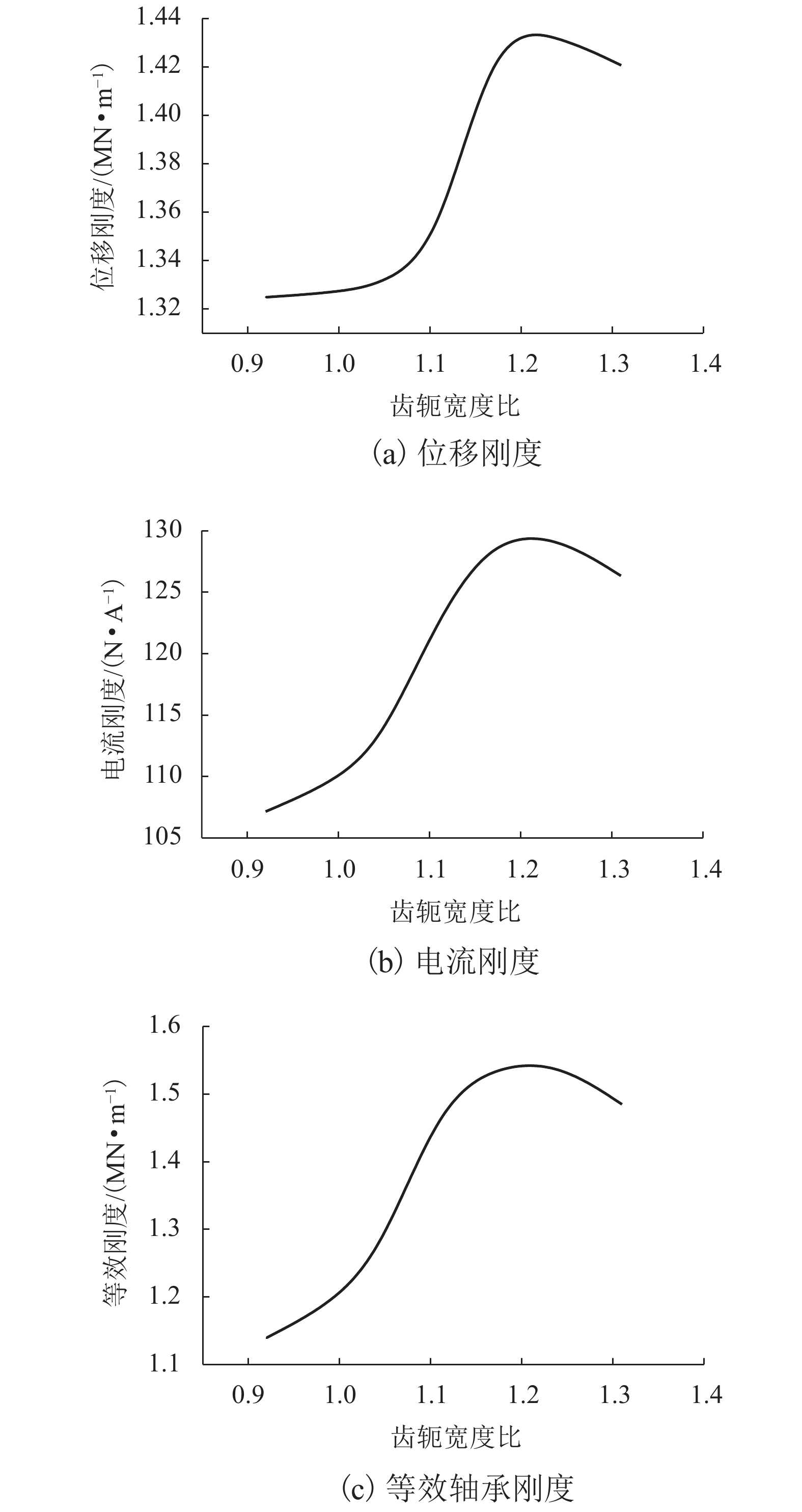
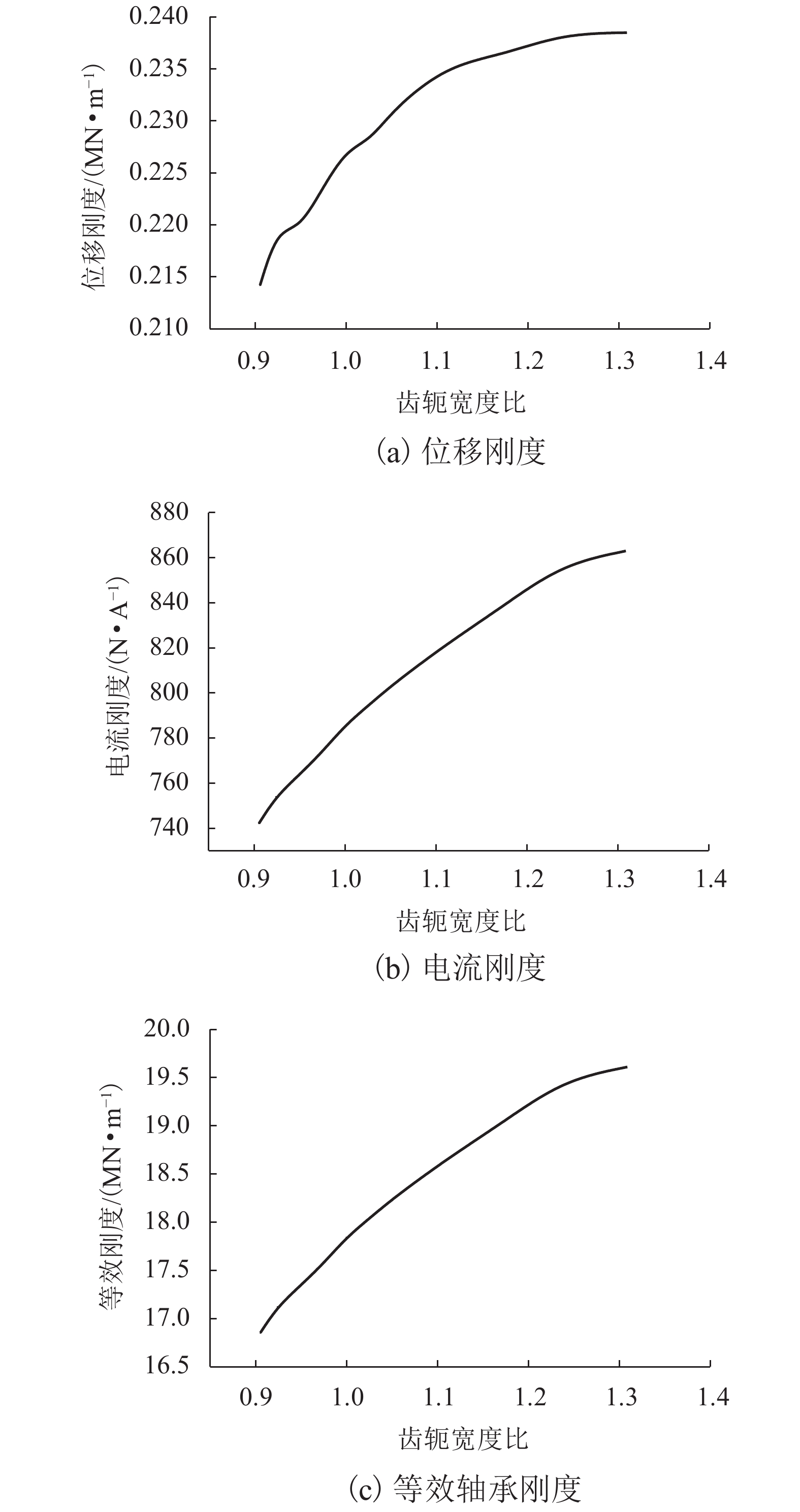
在位移x = 0,控制电流ib = 0附近,支承刚度与齿轭宽度比的关系如图10所示,随着齿轭宽度比增加,支承刚度近似线性增加. 在x = 0,ib = 4 A附近,支承刚度与齿轭宽度比的关系如图11所示,增加齿轭宽度比,支承刚度先增加后减小. 这是由于在ib = 0时,轴承定子磁感应强度较低,对λf基本无影响,增大齿轭宽度比可增加磁极面积,使得轴承支承刚度增加;但在ib = 4 A时,轴承定子磁感应强度较高,增大齿轭宽度比易导致轭部饱和,增大齿部面积的同时会导致λf减小,且减小幅度越来越大,使得支承刚度先增加后减小.
2.3 轴承结构确定
为保证轴承在最恶劣工况下仍可得到更高的支承刚度,在最大控制电流(接近饱和)下,确定满足支承刚度范围的最优齿轭宽度比. 具体规则为:首先确定最大控制电流下最大支承刚度对应齿轭宽度比,如此齿轭宽度比下的最大支承刚度(未饱和)不超过设计范围,则该齿轭宽度比即为优化结果,如已超过设计范围,则齿轭宽度比应取设计范围最大值kb1对应结果.
对于该轴承结构,最优齿轭宽度比为1.2,此时轴承在未饱和时最大支承刚度为1.92 × 107 N/m,不超过前述分析的支承刚度设计范围,同时在最恶劣工况即最大控制电流时可得到支承刚度最大值,较等磁路面积提高了25%.
3. 转子固有频率实验
分别进行了模态敲击实验和升频识别实验,对前述设计轴承的支承刚度进行测量,并验证转子的刚体模态频率是否满足压缩机运行要求.
将转子装入前述设计磁悬浮轴承支承的压缩机中,在转子上布置多组加速度传感器,转子长度为580 mm,转子质量20 kg,电机转子外经80 mm,转子结构及加速度传感器布置如图12所示. 将转子静态悬浮,用冲击力锤敲击转子,加速度传感器采集得到转子响应曲线,曲线峰值对应频率即为转子的模态频率,得到刚体模态频率为207 Hz. 前、后径向轴承结构及控制参数均相同,代入式(22)可以得到此时轴承的支承刚度为1.77 × 107 N/m.
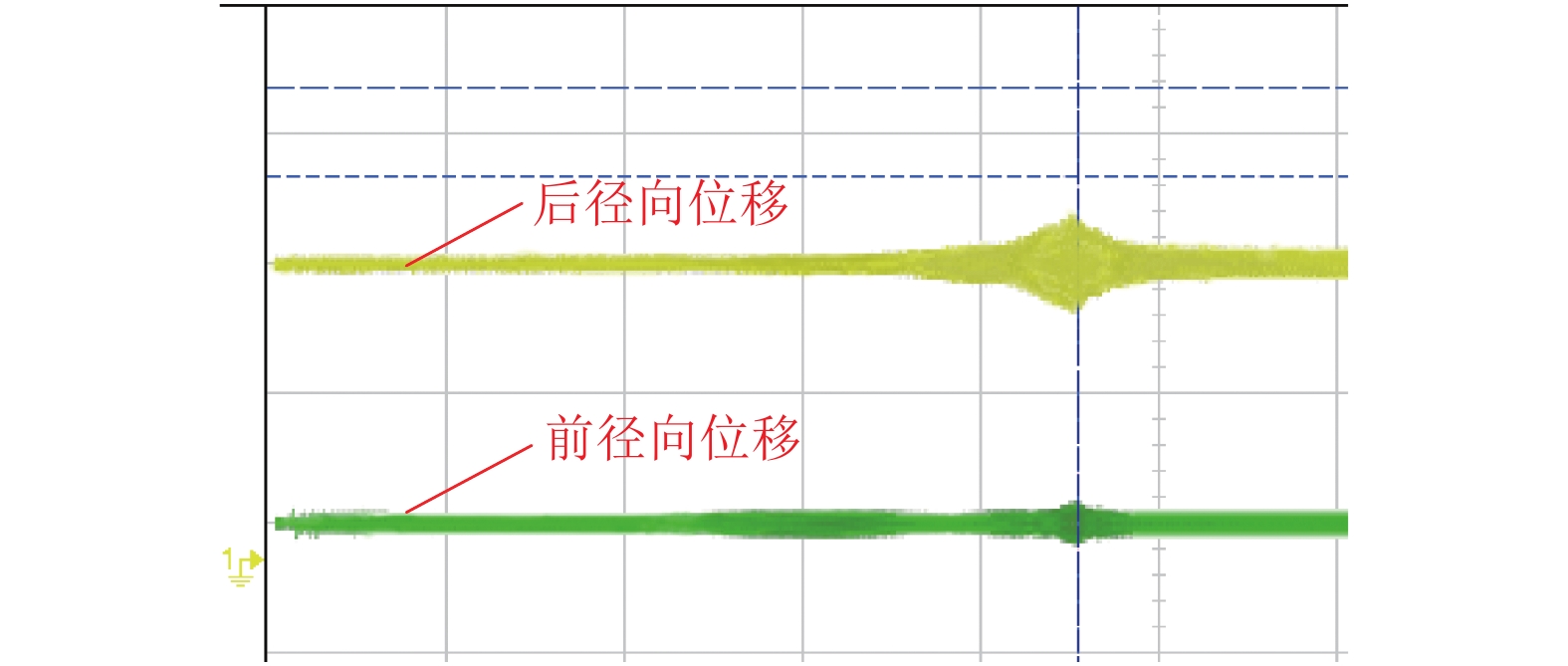
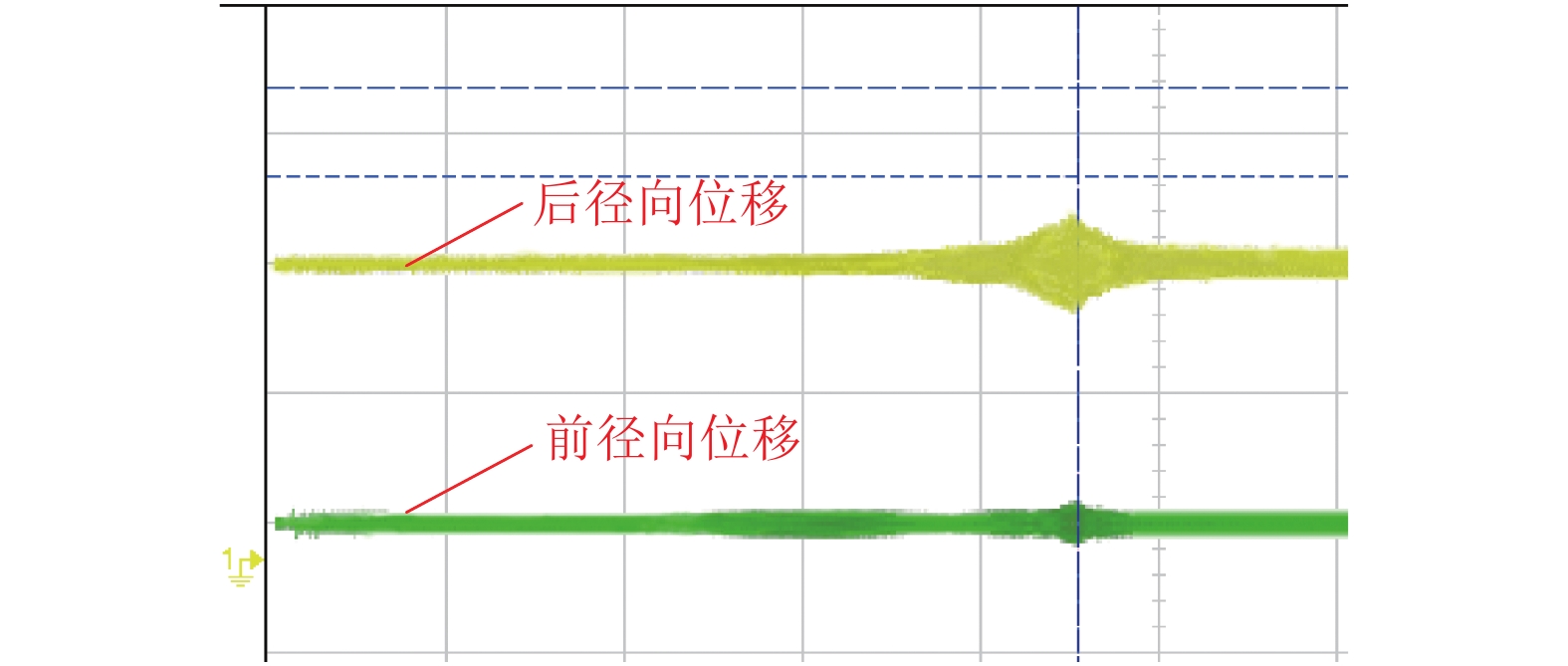
将转子装入压缩机后进行升频,升频过程中会经过刚体模态,如果不通过特殊控制方法,会出现轴承位移变差的情况,即轴承位移曲线会出现包络,如图13所示为该转子在压缩机中升频过程的位移曲线. 图中位移曲线包络处对应转子频率即为该支承刚度下的转子刚体模态频率,约为196 Hz. 代入式(22)可以得到此时轴承的支承刚度为1.68 × 107 N/m.
因此,通过模态敲击实验和升频识别实验测得转子刚体模态频率较接近,约为200 Hz,对应轴承支承刚度约为1.7 × 107 N/m,与仿真得到支承刚度最大值约10%偏差. 这是由于此时控制电流不为0,故而支承刚度略小于控制电流为0时的最大值.
转子工况运行频率范围为325~500 Hz,运行转速与刚体模态频率有60%以上的隔离裕度. 因此,采用该转子结构及支承参数,压缩机在工况运行区间可有效避免共振,保证转子运行稳定性.
4. 总 结
1) 本文从结构参数角度分析了磁悬浮轴承支承刚度的影响因素,提出不等磁路面积结构以优化支承刚度.
2) 针对制冷离心压缩机运行转速范围宽的特点,为保证其不发生共振并提高抗干扰能力,提出支承刚度设计准则,即在满足刚体模态共振隔离裕度的前提下,支承刚度设计应尽可能高.
3) 对应用于150 冷吨磁悬浮离心压缩机的主动式磁悬浮轴承采用该方法进行刚度优化设计,使其在最恶劣工况,即对应最大控制电流的情况下,支承刚度较常规等磁路面积结构提高了25%.
致谢:广东省高速节能电机系统重点实验室(ACSKL2018KT08)的支持.
-
-
[1] XU Y P, SHEN Q, ZHANG Y, et al. Dynamic modeling of the active magnetic bearing system operating in base motion condition[J]. IEEE Access, 2020, 8: 166003-166013. doi: 10.1109/ACCESS.2020.3022996 [2] KIM C S, JUNG H H, PARK B K. A study on the optimal design for a magnetic bearing-rotor with maximum stiffness using a genetic algorithm[J]. Journal of the Korean Society of Manufacturing Process Engineers, 2013, 12(6): 167-174. doi: 10.14775/ksmpe.2013.12.6.167 [3] YANG G J, XU Y, SHI Z G, et al. Characteristic analysis of rotor dynamics and experiments of active magnetic bearing for HTR-10GT[J]. Nuclear Engineering and Design, 2007, 237(12/13): 1363-1371. [4] JUNG H H, KANG S H, CHO B H, et al. A design technique for a magnetic bearing-rotor in a turbo blower considering critical speeds[J]. Advanced Materials Research, 2012, 569: 564-567. doi: 10.4028/www.scientific.net/AMR.569.564 [5] FUJIWARA H, YANAGIHARA K, MATSUSHITA O, et al. Design of active magnetic bearing rotors and their control method for passing through fourth bending critical speed[J]. Mechanical Engineering Journal, 2019, 6(3): 00481.1-00481.11. [6] 任正义,朱健国,杨立平. 基于ANSYS Workbench的飞轮转子临界转速计算分析[J]. 机械工程师,2019(9): 23-24,27.REN Zhengyi, ZHU Jianguo, YANG Liping. Calculation and analysis on critical speed of flywheel rotor based on ANSYS workbench[J]. Mechanical Engineer, 2019(9): 23-24,27. [7] 蒋启龙,胡振球. 轴向主动磁轴承的改进不完全微分PID控制[J]. 西南交通大学学报,2015,50(2): 241-246. doi: 10.3969/j.issn.0258-2724.2015.02.006JIANG Qilong, HU Zhenqiu. Improved incomplete derivative PID control of axial active magnetic bearing[J]. Journal of Southwest Jiaotong University, 2015, 50(2): 241-246. doi: 10.3969/j.issn.0258-2724.2015.02.006 [8] 黎松奇,张昆仑. 单磁铁悬浮系统自激振动的稳定性分析及抑制[J]. 西南交通大学学报,2015,50(3): 410-416. doi: 10.3969/j.issn.0258-2724.2015.03.004LI Songqi, ZHANG Kunlun. Self-excited vibration of single-magnet suspension system: stability analysis and inhibition[J]. Journal of Southwest Jiaotong University, 2015, 50(3): 410-416. doi: 10.3969/j.issn.0258-2724.2015.03.004 [9] CHITTLANGIA V, LIJESH K P, AKASH K, et al. Optimum design of an active magnetic bearing considering the geometric constraints[J]. Technology Letters, 2014, 1(3): 23-30. [10] LIJESH K P, HIRANI H. Optimization of eight pole radial active magnetic bearing[J]. Journal of Tribology, 2015, 137(2): 024502.1-024502.7. [11] 周瑾,高天宇,董继勇,等. 基于Isight的径向磁悬浮轴承结构优化设计[J]. 轴承,2018(7): 6-11.ZHOU Jin, GAO Tianyu, DONG Jiyong, et al. Optimal design for structure of radial magnetic bearings based on isight[J]. Bearing, 2018(7): 6-11. [12] KANG K, PALAZZOLO A. Homopolar magnetic bearing saturation effects on rotating machinery vibration[J]. IEEE Transactions on Magnetics, 2012, 48(6): 1984-1994. doi: 10.1109/TMAG.2012.2182776 [13] GERAMI A, ALLAIRE P, FITTRO R. Control of magnetic bearing with material saturation nonlinearity[J]. Journal of Dynamic Systems, Measurement, and Control, 2015, 137(6): 061002.1-061002.10. 期刊类型引用(1)
1. 金俊杰,王岩峰,徐程程,陆文轩,张晓友,孙凤,徐方超. 人工肾脏泵用磁悬浮轴承设计与磁力特性分析. 西南交通大学学报. 2024(04): 795-803 .  本站查看
本站查看其他类型引用(3)
-






 下载:
下载:






























































 下载:
下载: