Traffic Equilibrium Model of Reliable Network Based on Bounded Rationality
-
摘要:
为探索交通系统不确定性和出行者心理感知差异对出行路径选择行为的影响,将路网可靠性和有限理性融入出行者的路径选择决策中,提出双目标交通网络均衡模型. 为应对模型多解问题,建立出行可靠性和有限理性下的贝叶斯随机用户均衡模型,运用贝叶斯统计和双层规划框架估计权重系数,采用变分不等式刻画交通均衡模型;分别设计迭代算法(iterative algorithm,IA)和相继平均算法(method of successive average,MSA)求解贝叶斯权重系数估计和变分不等式交通网络均衡模型. 算例表明:随着观测变量和输入变量扰动变小,估计参数的均方根误差逐步减小;IA在运行15 s后均方根误差达到0.05,MSA在1 s内收敛精度达到10−6;变分不等式均衡模型可以同时反映出行者的风险态度和有限理性决策过程.
Abstract:To explore the influence of the uncertainties of traffic systems and travelers’ perception differences on route choice behavior, the bi-objective traffic network equilibrium model is proposed by introducing the network reliability and bounded rationality into travelers’ route choice decision process. To solve multiple solutions of bi-objective user equilibrium model, the Bayesian stochastic user equilibrium model considering travel time reliability and bounded rationality is built, where the Bayesian statistics and bi-level program framework are used to estimate the weight coefficients, and the variational inequality is adopted to build the traffic equilibrium model. The iterative algorithm (IA) and the method of successive average (MSA) are used for the Bayesian estimation model of weight coefficient and variational inequality traffic network equilibrium model, respectively. Case studies show that, the root mean square error (RMSE) of the estimated parameter is decreasing with the increasing of disturbances of observed data and input variable; RMSE reaches to 0.05 after running IA for 15 s, and the convergence accuracy of MSA reaches 10−6 within 1 s; the variational inequality equilibrium model explores traveler’s risk preference and bounded rational decision process.
-
Key words:
- traffic engineering /
- traffic equilibrium /
- reliability /
- bounded rationality /
- Bayesian
-
路径选择建模是交通工程师决策长期交通规划和短期交通管理的重要步骤,在给定道路网络结构和交通需求下,路径选择模型通过解析出行者路径选择机理来推导路段交通流量,同时对交通网络性能进行测试评估.
出行者的路径选择过程不仅受当前网络交通状况(外部因素)的影响,还受出行者自身感知异质性(内部因素)的影响[1]. 根据交通状况影响因素,许多学者采用出行时间和可靠性决策出行路径. Liu等[2]表明出行时间可靠性是交通系统性能中至关重要的影响指标. 在获知网络交通状况后,出行者根据自身感知来决策出行路径[3]. 出行者不会都选择时间最短的出行路径,通常可以接受比最短时间稍长一些的路径. 这是因为:1) 出行者缺乏对出行信息的完全认知;2) 路网系统的不确定性影响出行者做出最优决策;3) 根据以往出行经验,出行者存在惯性心理. Ramos等[4]通过一系列路径选择实验发现,由于出行惯性,在可接受时间延误范围内通勤者不会选择改变自己当前的路径到最短路径上. Zhu等[5]通过GPS和地理信息系统(GIS)数据发现,大部分出行者在选择路径时是有限理性的,他们不一定选择最短路径. Fayyaz等[6]也认为在路径选择过程中,比起出行时间,出行者更关心出行可靠性.
近年来,学者们对出行时间可靠性下的路径选择行为开展了研究. 部分研究把可靠性描述为出行计划中的迟到和早到惩罚;Watling[7]量化通勤出行中迟到惩罚分布,Zhu等[8]在出行效用中融入随机延误,并在弹性通勤中分析出行可靠性. 部分研究运用出行效用的均值和方差来描述出行可靠性;Zhang等[9]采用出行时间预算分析交通需求不确定性,Xu等[10]考虑出行时间偏见,建立弹性需求下的均值超量出行可靠性模型. 与此同时,可以采用鲁棒优化、惯性特征、风险分析、数据统计、网络扰动恢复力测试等方法构建出行时间可靠性下的路径选择模型[11].
由于有限理性可以更准确地描述出行者在路径选择过程中的心理,近几年逐渐成为交通均衡理论的研究热点. Di等[12-15]将有限理性引入到路径选择建模中,揭示了出行者并不会全都选择最短路出行,多于最短路径出行时间一定范围内的道路同样会被选择. Sun等[16]分析了出行者在有限理性出行中使用路径和非使用路径特征. Wang等[17]进一步将有限理性扩展到动态交通均衡.
尽管上述文献运用不同方法对路径选择模型进行了研究,但仍然存在一些不足:1) 传统的路径选择模型缺乏综合考虑出行者有限理性和出行时间可靠性;2) 存在的有限理性均衡模型均认为有限理性阈值是一个已知常数;3) 在建立出行时间和可靠性加权模型时,缺乏对参数估计方法的研究. 为此,本文同时考虑出行时间、出行可靠性和出行者的有限理性,提出双目标用户均衡方法,为了优化解的性质,进一步建立出行可靠性和有限理性下的贝叶斯随机用户均衡模型,设计迭代算法(iterative algorithm,IA)和相继平均算法(method of successive average,MSA)求解,并进行数值仿真实验.
1. 双目标用户均衡模型
在实际出行过程中,出行者往往会根据多个因素来决策出行路径,比如出行时间、出行可靠性等. 采用出行时间预算理论构建出行时间可靠性,数学模型如式(1)所示.
min{Rω,k(T)|P(Tω,k⩽(Eω,k(T)+Rω,k(T)))⩾α}, (1) 式中:
$E_{\omega ,k}^{}\left( {\boldsymbol{T}} \right)$ 、$R_{\omega ,k}^{}\left({\boldsymbol{T}} \right)$ 分别为OD (origin-destination)对$ \omega $ 第$ k $ 条路径的平均出行时间、可靠出行时间,分别如式(2)、(3)所示;$ \omega \in W $ ,$ W $ 为道路网络的OD对集;$ k \in K_\omega ^{} $ ,$ K_\omega ^{} $ 为OD对$ \omega $ 所有路径的集合;${\boldsymbol{T}} = \left( {T_{\omega ,k}^{}} \right)$ ,$ T_{\omega ,k}^{} $ 为OD对$ \omega $ 第$ k $ 条路径的出行时间;$ \alpha $ 为置信水平;$ P\left( {\text{•}} \right) $ 为概率函数.Eω,k(T)=∑aδa,ω,k[ta,0+bta,0vna(1−λ1−na)κna(1−λa)(1−n)], (2) Rω,k(T)=Φ−1(α)√∑aδa,ω,kb2t2a,0v2na[1−λ1−2naκ2na(1−λa)(1−2n)−(1−λ1−naκna(1−λa)(1−n))2], (3) 式中:
${\varPhi ^{{\text{ −}}1}}(\alpha )$ 为标准正态分布的累积分布反函数;${v_a}$ 为路段a上的交通流量,${\boldsymbol{v}} = \left( {{v_a}} \right)$ ,$a \in A$ ,$A$ 为路段集;$ t_{a,0}^{} $ 为路段a自由流速度;$ \delta _{a,\omega ,k}^{} $ 为路径、路段关联变量,当路径$ k $ 包含路段a时,$ \delta _{a,\omega ,k}^{} = 1 $ ,否则,$ \delta _{a,\omega ,k}^{} = 0 $ ;b、n为路段性能函数$ t_{a,0}^{}\left[ {1 + b{{\left( {{{v_a^{}} \mathord{\left/ {\vphantom {{v_a^{}} {{\kappa _a}}}} \right. } {{\kappa _a}}}} \right)}^n}} \right] $ 中的常数,通常$ b{\text{ = }}0.15 $ ,$ n{\text{ = }}4 $ ;$ {\kappa _a} $ 为路段a的容量上界;$ {\lambda _a} $ 为路段a的容量上界与下界的比例系数.同时,出行者本身并非完全理性,不一定选择耗时最短的路径,通常出行者可以接受出行时间比最短路稍微长一些的路径. 通常来说,有限理性阈值随着最短出行时间的增加而增加,路程越遥远,出行者可接受的出行时间偏差越大. 同时,该阈值存在一个上界,因为没有出行者可以忍受一个非常大的时间偏差. OD对
$\; \omega $ 的有限理性阈值$ {\varepsilon _\omega }\left( {\text{•}} \right) $ 如式(4)所示,其曲线如图1所示.εω(T)=εmax(1−1exp(σminkEω,k(T))), (4) 式中:
$ \sigma $ 为用户关于最短出行时间的敏感性系数,其值越大,用户对最短出行时间越敏感;$ {\varepsilon _{\max }} $ 为有限理性阈值的上界.根据出行者出行特征,提出了双目标用户均衡模型,即最小化平均出行时间及出行阈值之和、最小化选择路径的可靠出行时间,如式(5)所示.
{min{Eω,k(T)+εω(T)},minRω,k(T). (5) 2. 出行可靠性和有限理性下的贝叶斯随机用户均衡模型
2.1 多目标出行阻抗
双目标用户均衡模型通常具有多个解,可采用权重法、约束法、动态规划等方法获取双目标模型的帕累托前沿解集,如通过调整权重法的权重系数便可获得模型的最优解集. 从帕累托前沿解集中抓取出与实际居民出行状况最接近的解(交通网络流状态),是城市交通网络规划的基本需求. 由于贝叶斯估计能够同时利用历史经验和当前信息,因此,本文设计了基于贝叶斯统计的权重法来抓取双目标用户均衡模型的最优交通网络流状态. 定义OD对
$ \omega $ 第$ k $ 条路径的多目标出行阻抗为cω,k=Eω,k(T)+εω(T)+βωRω,k(T), (6) 式中:
$ {\beta _\omega } $ 为OD对$ \omega $ 的权重系数,${\boldsymbol{\beta}} = \left( {{{{\beta}} _\omega }} \right)$ .提出的多目标出行阻抗组合了平均出行时间、可靠出行时间和出行者的有限理性,因此,可以更全面地描述了出行者路径选择行为的过程.
2.2 贝叶斯权重系数估计
考虑测量误差e,假设e服从正态分布. 根据城市交通网络流理论,路段流量和出行需求之间的转换关系如式(7)所示.
va=∑ω∑kpω,kδa,ω,kqω+ea, (7) pω,k=exp(−θcω,k)∑kexp(−θcω,k), (8) 式中:
$ {e_a} $ 为路段a上的测量误差,${\boldsymbol{e}} = \left( {{e_a}} \right)$ ;$q_\omega ^{}$ 为OD对$ \omega $ 的交通需求量;$ p_{\omega ,k}^{} $ 为OD对$ \omega $ 路径$ k $ 上的出行者路径选择概率;$\theta $ 为出行用户感知误差偏差系数.运用多元正态分布(multivariate normal distribution)描述交通小区的权重系数,并且运用观测路段流量估计权重时,式(8)的分母保持常数. 因此,观测变量与权重之间的关系可以用式(7)、(8)来表达. 随着观测变量的融入,待估变量的后验概率服从多元正态分布[18].
${\boldsymbol{\beta }}$ 的均值向量${{\boldsymbol{\mu }}_{{\boldsymbol{Y}}\left| {{\boldsymbol{Z}} = {\boldsymbol{z}}} \right.}}$ 和变量之间的协方差矩阵${{\boldsymbol{\varSigma }}_{{\boldsymbol{Y}}\left| {{\boldsymbol{Z}} = {\textit{z}}} \right.}}$ 分别如式(9)、(10)所示.μY|Z=z=μY+ΣYZΣ−1ZZ(z−μZ), (9) ΣY|Z=z=ΣYY−ΣYZΣ−1ZZΣZY, (10) 式中:Y为所有未观测变量向量(本文中的权重系数);Z为所有观测变量向量,z为其观测值;
${{\boldsymbol{\mu }}_{\boldsymbol{Y}}}$ 、${{\boldsymbol{\mu }}_{\boldsymbol{Z}}}$ 分别为变量Y、Z的均值向量;${{\boldsymbol{\varSigma }}_{{\boldsymbol{YZ}}}}$ 为未观测变量Y和观测变量Z之间的协方差矩阵;${{\boldsymbol{\varSigma }}_{{\boldsymbol{ZY}}}}$ 、${{\boldsymbol{\varSigma }}_{{\boldsymbol{YY}}}}$ 、${\boldsymbol{\varSigma }}_{{\boldsymbol{ZZ}}}^{}$ 均为对应的协方差矩阵.根据观测道路流量值,设计贝叶斯权重系数估计双层模型.
上层模型:
argmaxβfω,k(Z|β)P(β)∫ω,kfω,k(Z|β)P(β)dβ, (11) 约束为式(7)、(8);
下层模型:
fω,k=qωpω,k=qωP(cω,k⩽cω,l,∀l∈Kω,l≠k|cω),∀l∈Kω, (12) 式(11)、(12)中:
$ f_{\omega ,k}^{} $ 为OD点对$ \omega $ 上第$ k $ 条路径上的流量;${\boldsymbol{c}}_\omega ^{}$ 为OD点对$ \omega $ 上所有路径的多目标出行阻抗向量,即${\boldsymbol{c}}_\omega ^{} = \left( {c_{\omega ,k}^{}} \right)$ .双层规划模型的上层运用贝叶斯估计获取权重系数
$ {\beta _\omega } $ ,下层推导出行者路径选择概率$ p_{\omega ,k}^{} $ .2.3 均衡模型及解的性质
考虑一个强连通交通网络,分析出行可靠性和有限理性下的贝叶斯随机用户均衡模型状态.
定义1 在出行可靠性和有限理性下的贝叶斯随机用户均衡状态下,仅改变自己的出行路径,出行者不可能减小自身的感知多目标出行阻抗.
式(12)的均衡条件可以等价于:探索到一个路径流量解
${{\boldsymbol{f}}^ * } \in \varOmega$ ,使得对于$\forall {{\boldsymbol{f}}^ * } \in \varOmega$ ,均满足式(13)所示的变分不等式;$ \varOmega $ 为模型解集.[c(f∗)+(1/θ)lnf∗]T(f−f∗)⩾0,∀f∈Ω, (13) Ω={f|Λf=q,f⩾0,q⩾0}, (14) 式中:
${\boldsymbol{f}} = {\text{(}}f_{\omega ,k}^{}{\text{)}}$ ;$ {\boldsymbol{c}}\left( {\text{•}} \right) $ 为所有OD点对上所有路径的多目标出行阻抗向量;$ {{\boldsymbol{f}}^ * } $ 为路径流量均衡解;$ {\boldsymbol{\varLambda} }$ 为路径、路段的关联矩阵,${\boldsymbol{\varLambda}} = (\delta _{a,\omega ,k}^{})$ ;${\boldsymbol{q}} = (q_\omega ^{})$ .式(13)、(14)解的存在性和等价性如定理1、定理2所示.
定理1 (存在性) 出行可靠性和有限理性下的贝叶斯随机用户均衡模型,即式(13)、(14)至少有一个解.
证明 可行集即式(14)是非空、有界闭凸集,且
$E_{\omega ,k}^{}\left( {\boldsymbol{T}} \right)$ 、$\varepsilon _\omega ^{}\left( {\boldsymbol{T}} \right)$ 和$R_{\omega ,k}^{}\left( {\boldsymbol{T}} \right)$ 均是关于${\boldsymbol{f}}$ 的连续函数,因此,式(13)、(14)至少存在一个解. 证毕.定理2 (等价性) 式(13)、(14)的解与所建模型的均衡解是等价的.
证明 式(13)、(14)等价于
${\left[ {{\boldsymbol{c}}\left( {{{\boldsymbol{f}}^ * }} \right) + \left( {{1 \mathord{\left/ {\vphantom {1 \theta }} \right. } \theta }} \right)\ln {{{\boldsymbol{f}}^ * }} } \right]^{\rm{T}}}{\boldsymbol{f}} \geqslant {\left[ {{\boldsymbol{c}}\left( {{{\boldsymbol{f}}^ * }} \right) + \left( {{1 \mathord{\left/ {\vphantom {1 \theta }} \right. } \theta }} \right)\ln \;{{{\boldsymbol{f}}^ * }} } \right]^{\rm{T}}}{{\boldsymbol{f}}^ * },\;\;\forall {\boldsymbol{f}} \in \varOmega$ ,因此,${{\boldsymbol{f}}^ * }$ 是变分不等式的均衡解,当且仅当${{\boldsymbol{f}}^ * }$ 是式(15)的最优解.minf∈Ω[c(f∗)+(1/θ)lnf∗]Tf. (15) 考虑式(15)的KKT (Karush-Kuhn-Tucker)条件,可以得到:
f∗ω,k[cω,k(f∗)+(1/θ)lnf∗ω,k−˜πω]=0, (16) cω,k(f∗)+(1/θ)lnf∗ω,k−˜πω⩾0, (17) f∗ω,k⩾0, (18) 式中:
$ f_{\omega ,k}^ * $ 为OD对$ \omega $ 第$ k $ 条路径的流量均衡解;$ \tilde \pi _\omega ^{} $ 为OD对$ \omega $ 的乘子.由式(16)~(18)发现,变分不等式(式(13)、式(14))的解与均衡条件(式(12))是等价的.
证毕.
3. 模型算法
在求解贝叶斯参数估计时,运用IA算法反复计算上、下层模型,其中,上层的贝叶斯最大后验概率估计运用式(9)、(10)求解,下层模型通过MSA算法计算. 由于所建立的出行可靠性和有限理性下的贝叶斯随机用户均衡模型中多目标出行阻抗非可加,因此,使用路径算法求解.
算法1 IA算法求解贝叶斯参数估计.
步骤1 初始化:收敛精度
$ \zeta $ 、测量误差e、权重系数${\boldsymbol{\beta }}$ 先验均值向量(同时设初始权重系数值为${{\boldsymbol{\beta}} ^{\left( 1 \right)}}$ )和变量之间的协方差矩阵、观测路段流量Z. 设置初始迭代步数d1=1,下文中,参数上标(d1)表示参数值的第d1次迭代.步骤2 求解下层SUE模型:通过MSA算法求解下层模型,获得关联系数、出行时间均值、方差和有限理性阈值.
步骤3 求解上层贝叶斯参数估计模型:根据式(9)、(10),使用观测路段流量计算权重系数过渡值
${{\boldsymbol{\bar \beta }}^{\left( {{d_1}} \right)}}$ .1) 设置观测路段编号s,获取观测路段集维度smax.
2) 根据观测的路段流量zs,运用式(9)、(10)更新权重系数的后验均值
${{\boldsymbol{\mu}}_{{\boldsymbol{Y}}\left| {{\boldsymbol{Z}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}} \right.}}$ 和变量之间的协方差矩阵${{\boldsymbol{\varSigma }}_{{\boldsymbol{Y}}\left| {{\boldsymbol{Z}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}} \right.}}$ ,令观测变量均值${{\boldsymbol{\mu}} _{{\boldsymbol{Z}}\left| {{\boldsymbol{Z}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}} \right.}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}$ ,观测变量间的协方差矩阵${{\boldsymbol{\varSigma}} _{{\boldsymbol{Z}}\left| {{\boldsymbol{Z}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}} \right.}} = {\boldsymbol{0}}$ ,${{\mathbf{\mu }}^{\left( s \right)}} = {{\mathbf{\mu }}_{{\boldsymbol{Y}}\left| {{\boldsymbol{Z}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}} \right.}}$ ,${{\boldsymbol{\varSigma }}^{\left( s \right)}} = {{\boldsymbol{\varSigma }}_{{\boldsymbol{Y}}\left| {{\boldsymbol{Z}} = {{\boldsymbol{z}}_{\boldsymbol{s}}}} \right.}}$ .3) 收敛检验:令
$ s = s + 1 $ ,如果$ s \geqslant {s_{\max }} $ ,停止计算,令权重系数过渡值${{\boldsymbol{\bar \beta }}^{\left( {{d_1}} \right)}}$ 等于更新后的权重均值${{\mathbf{\mu }}^{\left( s \right)}}$ ;否则转到2).步骤4 更新权重系数:令
${{\boldsymbol{\beta }}^{\left( {{d_1} + 1} \right)}} ={{\boldsymbol{\beta }}^{\left( {{d_1}} \right)}} + \left( {{{{\boldsymbol{\bar \beta }}}^{\left( {{d_1}} \right)}} - {{\boldsymbol{\beta }}^{\left( {{d_1}} \right)}}} \right) {{ \mathord{\left/ {\vphantom {1 {{d_1}}}} \right. } {{d_1}}}} $ .步骤5 收敛检验:如果
$\left\| {{{\boldsymbol{\beta }}^{\left( {{d_1} + 1} \right)}} - {{\boldsymbol{\beta }}^{\left( {{d_1}} \right)}}} \right\|\Big/\left\| {{{\boldsymbol{\beta }}^{\left( {{d_1}} \right)}}} \right\| \leqslant \zeta$ ,停止计算,${{\boldsymbol{\beta}} ^{\left( {{d_1}} \right)}}$ 就是最优的权重系数;否则,令$ {d_1} = {d_1} + 1 $ ,转到步骤2.根据最优权重系数值
${{\boldsymbol{\beta}} ^{\left( {{d_1}} \right)}}$ ,运用算法2求解出行可靠性和有限理性下的贝叶斯随机用户均衡模型.算法2 通过MSA算法求解出行可靠性和有限理性下的贝叶斯随机用户均衡模型.
步骤1 初始化:收敛精度
$ \nu $ ,权重系数${\boldsymbol{\beta}}$ ,可靠出行时间参数$ \alpha $ 、$ {\kappa _a} $ 、$ \theta $ ,有限理性参数$ {\omega _{\max }} $ 、$ \sigma $ ;设置初始迭代步数$ {d_2} = 1 $ . 根据路段流量计算多目标出行阻抗,即式(2)~(4)、(6),当$ {d_2} = 1 $ 时,路段流量设为0. 根据式(8)计算出行者路径选择概率,并运用式(12)进行流量加载,获得初始路径流量${{\boldsymbol{f}}^{\left( 1 \right)}}$ .步骤2 更新多目标出行阻抗:根据式(2)~(4)、式 (6)更新多目标出行阻抗
$ c_{\omega ,k}^{\left( {{d_2}} \right)} $ .步骤3 搜索方向:根据多目标出行阻抗
$c_{\omega ,k}^{\left( {{d_2}} \right)}$ ,运用式(8)、(12)加载道路流量,计算出辅助路径流量${{\boldsymbol{\bar f}}^{\left( {{d_2}} \right)}}$ .步骤4 更新路径流量:根据当前路径流量
${{\boldsymbol{f}}^{\left( {{d_2}} \right)}}$ 和辅助路径流量${{\boldsymbol{\bar f}}^{\left( {{d_2}} \right)}}$ ,计算搜索方向${{\boldsymbol{\bar f}}^{\left( {{d_2}} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}$ ;然后计算新的路径流量${{\boldsymbol{f}}^{\left( {{d_2} + 1} \right)}} = {{\boldsymbol{f}}^{\left( {{d_2}} \right)}} + {{\left( {{{{\boldsymbol{\bar f}}}^{\left( {{d_2}} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right)} \mathord{\left/ {\vphantom {{\left( {{{{\boldsymbol{\bar f}}}^{\left( {{d_2}} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right)} {{d_2}}}} \right. } {{d_2}}}$ .步骤5 收敛检验:如果
$\left\| {{{\boldsymbol{f}}^{\left( {{d_2} + 1} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right\|\Big/\left\| {{{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right\| \leqslant \nu$ ,停止计算,$f_{\omega ,k}^{\left( {{d_2}} \right)}$ 就是最优的路径流量;否则,令$ {d_2} = {d_2} + 1 $ ,转到步骤2.4. 算例分析
4.1 Nguyen-Dupuis网络
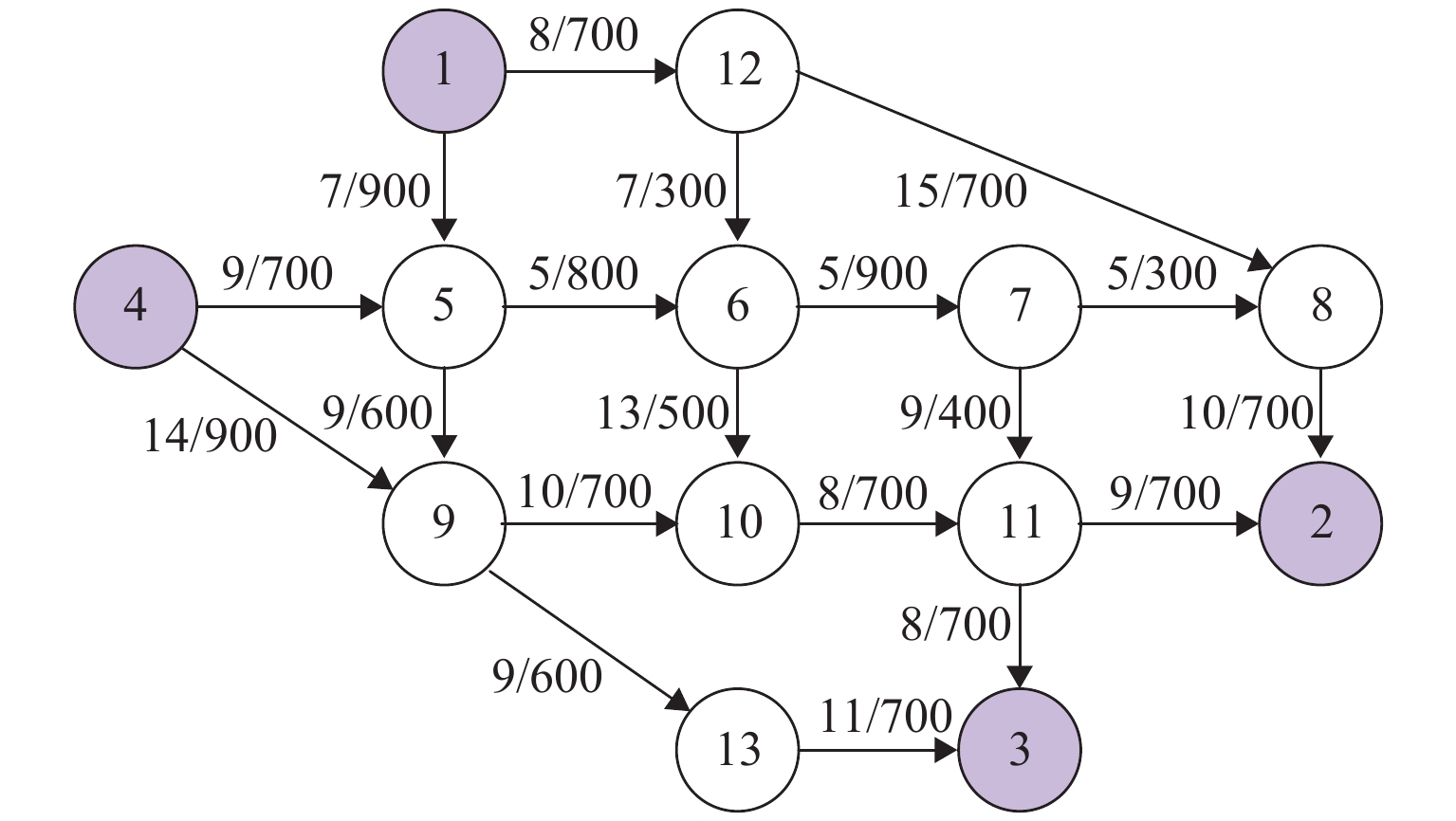
Nguyen-Dupuis网络属性如图2所示,OD对1—2、1—3、4—2、4—3的OD量分别为400、800、600、200辆. 图中:圈中数字为节点编号;有向线段上的数值分别为自由流旅行时间(单位:min)和道路容量(单位:辆/h),例如:节点1—12上的数字“8/700”表示路段自由流旅行时间为8 min,道路容量为700辆/h. 初始化模型中的各参数值:路段容量上界与下界的比例系数
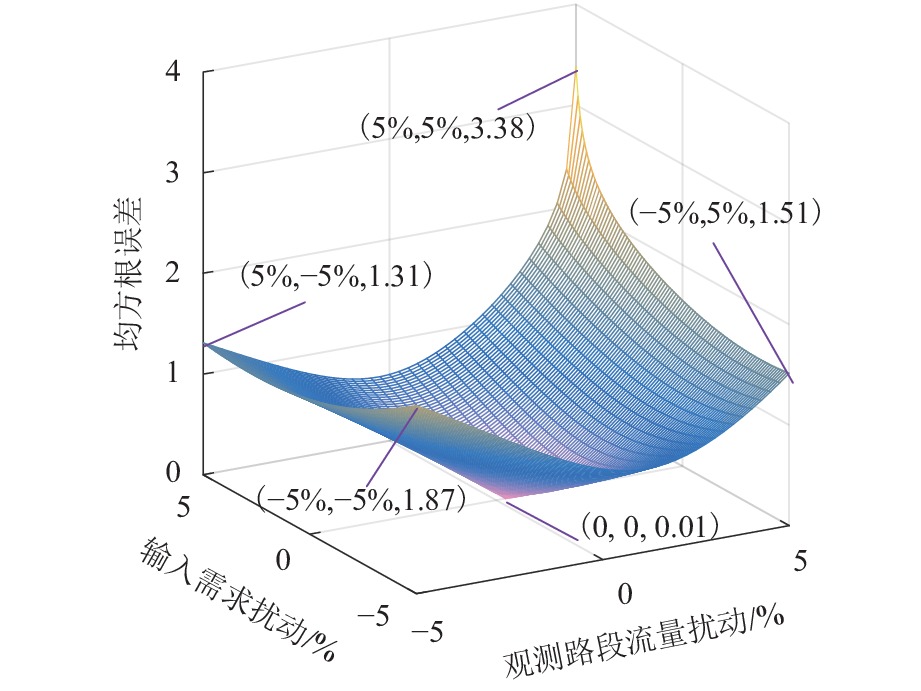
$ {\lambda _a} = 0.8 $ ;有限理性阈值上界$ {\varepsilon _{\max }}{\text{ = 1}} $ ;敏感系数$ \sigma = 0.02 $ ;置信水平$ \alpha = 0.08 $ ;出行用户感知误差偏差系数$\theta = 1$ ;权重系数先验均值向量为(0.25,0.25,0.25,0.25);变量之间的协方差矩阵为diag(0.5,0.5,0.5,0.5).通过计算并分析权重系数的灵敏度,图3测试了贝叶斯权重系数估计的鲁棒性,权重系数的评价指标为
eRMSE=‖ (5) 式中:eRMSE为均方根误差(root mean square error,RMSE);
${{\boldsymbol{\beta }}_{{\text{tru}}}}$ 、${{\boldsymbol{\beta }}_{{\text{est}}}}$ 分别为准确的和估计的权重系数向量;$ \left| \omega \right| $ 为OD对$ \omega $ 的路径数.为了说明建立模型的有效性,使用(2.00,1.50, 2.50,3.00)作为测试网络的“准确权重系数”. 可以发现,在输入需求和观测路段流量扰动都接近0时,估计参数的RMSE达到最小值0.01,表明提出的贝叶斯权重系数估计模型所估计的权重系数值与实际路径选择结构接近. RMSE随着输入需求和观测路段流量扰动的增加而增大,因此,输入参数(路段流量和交通需求)的扰动对权重系数值的估计产生显著影响.
进一步代入估计的权重系数值,对出行可靠性和有限理性下的贝叶斯随机用户均衡模型求解,结果如表1所示. 同上述理论一致,计算得到的解满足均衡条件:仅改变自己的出行路径,出行者不可能减小自身的感知多目标出行阻抗. 例如,在OD对1—2中的第1条路径(即1—12—8—2),根据式(4)、(8)、(12),得到有限理性阈值、选择概率和流量分别为
${\varepsilon _{1 - 2}}= 15[ 1 - 1 / \exp ( 0.02 \times 35.94 ) ] =7.69$ min,$ p_{1 - 2,1}^{} = $ $\exp [ - ( 35.94 + 7.69 + 2 \times 0.52 ) ] \Bigg/{\displaystyle\sum\limits_{k \in {K_{1 \,-\, 2}}} {{\text{exp}}\left( { \;-\; c_{1 \,-\, 2,k}^{}} \right)} }$ = 0.92,$ f_{1 - 2,1}^{} = p_{1 - 2,1}^{} {q_{1 - 2}} = 0.920\;6 \times 400 = 368.24 $ 辆,与模型的均衡条件一致.表 1 算例1的模型均衡解Table 1. Equilibrium results of proposed model for case 1OD 路径 时间均值/min 有限理
性阈值/min权重
系数可靠出
行时间/min感知多目标出行阻抗均值/min 流量/辆 1—2 1—12—8—2 35.94 7.69 2.00 0.52 44.67 368.24 1—5—6—7—8—2 38.36 0.70 47.45 23.02 1—5—6—7—11—2 40.35 0.72 49.48 3.02 1—5—6—10—11—2 43.77 0.25 51.96 0.25 1—5—9—10—11—2 44.41 0.20 52.50 0.15 1—12—6—7—8—2 40.04 0.66 49.05 4.65 1—12—6—7—11—2 42.04 0.67 51.07 0.61 1—12—6—10—11—2 45.46 0.07 53.29 0.07 1—3 1—5—6—7—11—3 39.58 8.20 1.50 0.72 48.86 327.15 1—5—6—10—11—3 43.01 0.26 51.60 21.28 1—5—9—10—11—3 43.65 0.21 52.17 12.07 1—12—6—7—11—3 41.27 0.68 50.49 64.53 1—12—6—10—11—3 44.69 0.10 53.04 5.04 1—5—9—13—3 39.85 0.46 48.74 369.93 4—2 4—5—6—7—8—2 39.66 8.21 2.50 0.67 49.55 302.33 4—5—6—7—11—2 41.66 0.69 51.60 39.21 4—5—6—10—11—2 45.08 0.18 53.74 4.66 4—9—10—11—2 41.41 0.05 49.75 250.74 4—5—9—10—11—2 45.72 0.09 54.16 3.05 4—3 4—5—6—7—11—3 40.89 7.82 3.00 0.70 50.81 1.40 4—5—6—10—11—3 44.31 0.19 52.70 0.21 4—5—9—10—11—3 44.95 0.11 53.10 0.14 4—9—13—3 36.84 0.42 45.92 184.53 4—5—9—13—3 41.15 0.42 50.23 2.43 4—9—10—11—3 40.64 0.08 48.70 11.30 4.2 Sioux Falls网络
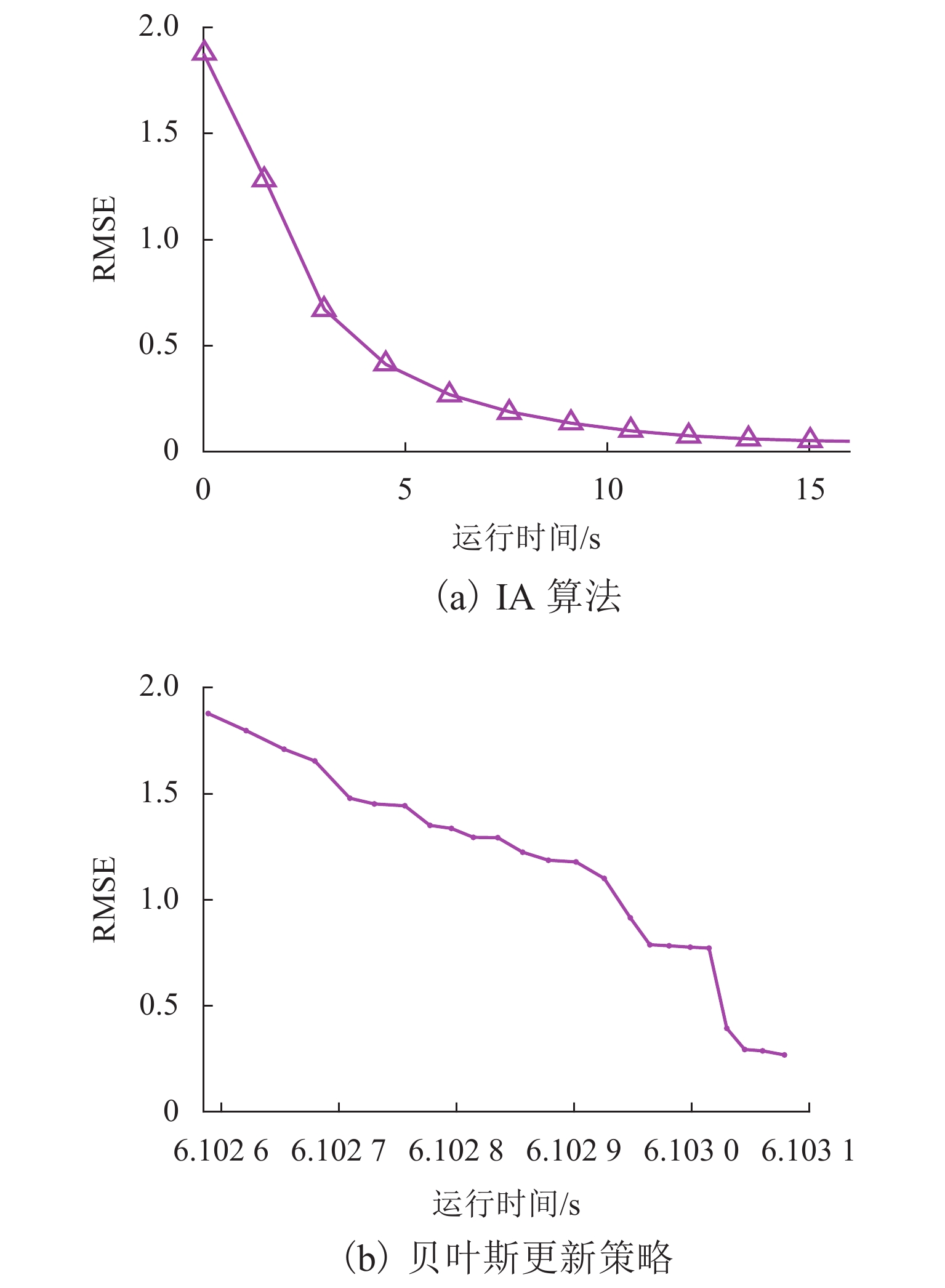
进一步将模型运用在Sioux Falls网络中,该路网由24个结点、76个路段和550个OD对组成,路段特性和OD需求见文献[19],其余参数设置与Nguyen-Dupuis网络算例一致. 图4绘制了IA算法和贝叶斯更新策略的收敛过程,其中,贝叶斯更新策略为IA算法的第5次迭代过程. 可以发现:运行15 s后,RMSE到0.05;迭代过程中权重系数的RMSE值稳定下降,表明算法具有良好的鲁棒性.
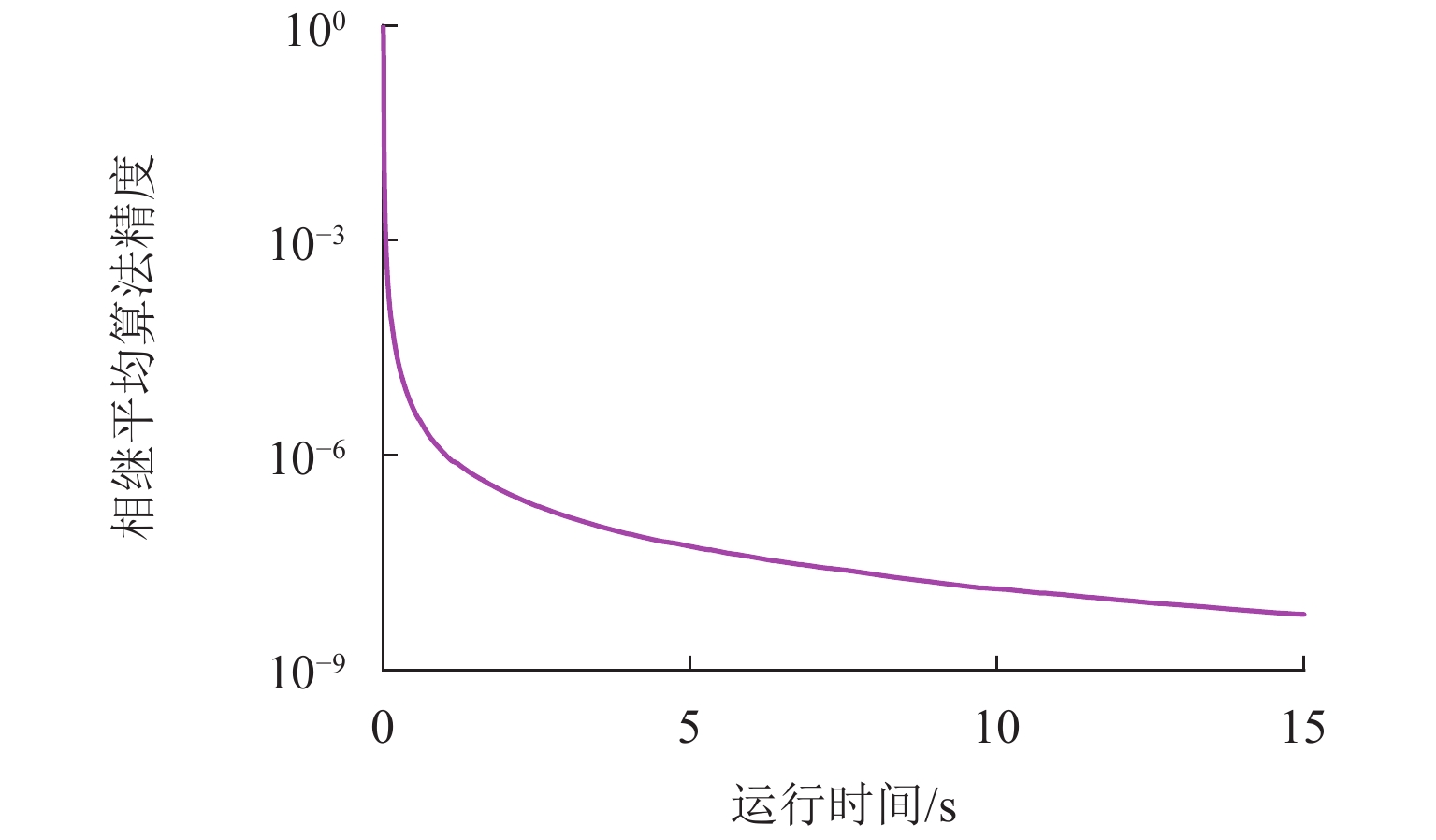
图5测试了求解出行可靠性和有限理性下的贝叶斯随机用户均衡模型算法的收敛速度,可以发现:设计的MSA算法能够快速收敛,在1 s内精度可达到10−6.
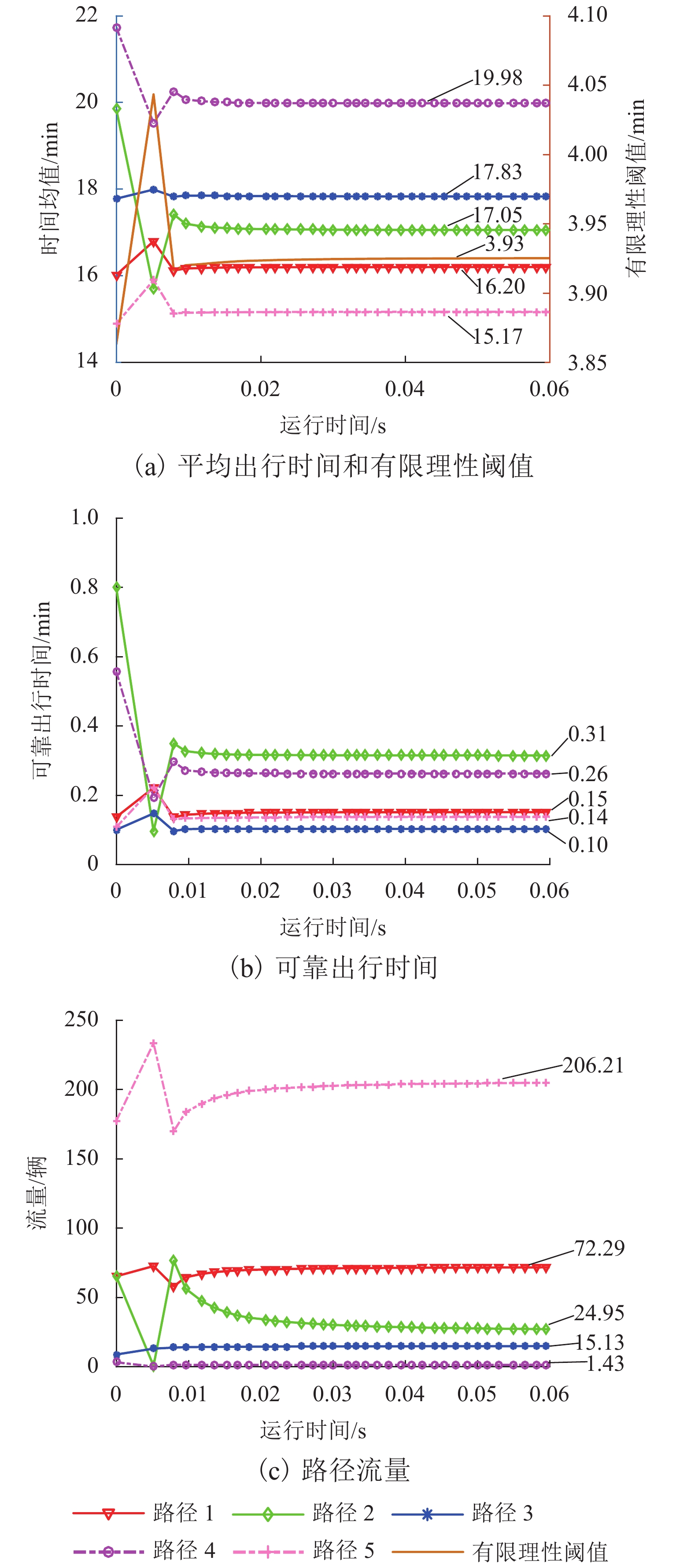
选取OD对11—6的5条路径(路径1~5)作为测试对象,图6展示了模型平均出行时间、有限理性阈值、可靠出行时间和路径流量的迭代过程和快速收敛性;同时可以验证算法收敛至模型均衡解,例如,在OD对11—6的第1条路径中,根据式(4)、式(8)、(12),计算出有限理性阈值、选择概率和路径流量分别为
$ {\varepsilon _{11 - 6}} = 15\left[ {1 - {1 \mathord{\left/ {\vphantom {1 {\exp \left( {0.02 \times 15.17} \right)}}} \right. } {\exp \left( {0.02 \times 15.17} \right)}}} \right] = 3.93 $ min,$p_{11 - 6,1}^{} = \exp \left[ { - \left( {16.20 + 3.93 + 1.3 \times 0.15} \right)} \right] \left/ \right.$ ${{} \mathord{ {\vphantom {{} {\sum\limits_{k \in {K_{11 - 6}}} {{\text{exp}}\left( { - c_{11 - 6,k}^{}} \right)} }}} } {\displaystyle\sum\limits_{k \in {K_{11 - 6}}} {{\text{exp}}\left( { - c_{11 - 6,k}^{}} \right)} }} = 0.26$ ,$ f_{11 - 6,1}^{} = p_{11 - 6,1}^{}{q_{11 - 6}} = 0.225\;9\times 320 =72.29 $ 辆. 因此,模型解收敛至均衡条件.5. 结 论
1) 出行者会同时根据出行时间、出行可靠性和自身的有限理性来选择出行路径,从而提出了双目标用户均衡模型. 在目标一中,用户追求有限理性范围内出行时间最短;在目标二中,用户同时期望最短的可靠出行时间. 为克服双目标模型多解的问题,进一步运用变分不等式建立了出行可靠性和有限理性下的贝叶斯随机用户均衡模型,该模型通过添加权重系数来统一化两个出行目标,并采用基于双层规划框架的贝叶斯方法估计权重系数. 分别设计了IA算法和MSA算法计算贝叶斯参数估计和变分不等式模型.
2) 数值实验表明,提出的贝叶斯权重系数估计模型能够推断精度较高且具有鲁棒性的权重系数值;输入参数的扰动对权重系数估计值产生显著影响,当扰动都接近0的时候,权重系数的均方根误差达到最小值0.01;设计的IA算法和MSA算法具有良好的鲁棒性,且可以快速收敛于均衡解,IA算法在运行15 s后均方根误差达到0.05,MSA算法在1 s时精度达到10−6.
3) 后续将在实际城市路网中进行模型应用,为交通规划提供数据参考;同时进一步将模型应用到拥堵收费、车辆限速等网络设计问题上.
-
表 1 算例1的模型均衡解
Table 1. Equilibrium results of proposed model for case 1
OD 路径 时间均值/min 有限理
性阈值/min权重
系数可靠出
行时间/min感知多目标出行阻抗均值/min 流量/辆 1—2 1—12—8—2 35.94 7.69 2.00 0.52 44.67 368.24 1—5—6—7—8—2 38.36 0.70 47.45 23.02 1—5—6—7—11—2 40.35 0.72 49.48 3.02 1—5—6—10—11—2 43.77 0.25 51.96 0.25 1—5—9—10—11—2 44.41 0.20 52.50 0.15 1—12—6—7—8—2 40.04 0.66 49.05 4.65 1—12—6—7—11—2 42.04 0.67 51.07 0.61 1—12—6—10—11—2 45.46 0.07 53.29 0.07 1—3 1—5—6—7—11—3 39.58 8.20 1.50 0.72 48.86 327.15 1—5—6—10—11—3 43.01 0.26 51.60 21.28 1—5—9—10—11—3 43.65 0.21 52.17 12.07 1—12—6—7—11—3 41.27 0.68 50.49 64.53 1—12—6—10—11—3 44.69 0.10 53.04 5.04 1—5—9—13—3 39.85 0.46 48.74 369.93 4—2 4—5—6—7—8—2 39.66 8.21 2.50 0.67 49.55 302.33 4—5—6—7—11—2 41.66 0.69 51.60 39.21 4—5—6—10—11—2 45.08 0.18 53.74 4.66 4—9—10—11—2 41.41 0.05 49.75 250.74 4—5—9—10—11—2 45.72 0.09 54.16 3.05 4—3 4—5—6—7—11—3 40.89 7.82 3.00 0.70 50.81 1.40 4—5—6—10—11—3 44.31 0.19 52.70 0.21 4—5—9—10—11—3 44.95 0.11 53.10 0.14 4—9—13—3 36.84 0.42 45.92 184.53 4—5—9—13—3 41.15 0.42 50.23 2.43 4—9—10—11—3 40.64 0.08 48.70 11.30 -
[1] 刘志伟,刘建荣,邓卫. 考虑潜在类别的市内机动化出行行为模型[J]. 西南交通大学学报,2021,56(1): 131-137.LIU Zhiwei, LIU Jianrong, DENG Wei. Inclusion of latent class in behavior model of motorized travel in city[J]. Journal of Southwest Jiaotong University, 2021, 56(1): 131-137. [2] LIU W, LI X W, ZHANG F N, et al. Interactive travel choices and traffic forecast in a doubly dynamical system with user inertia and information provision[J]. Transportation Research Part C: Emerging Technologies, 2017, 85: 711-731. doi: 10.1016/j.trc.2017.10.021 [3] WU L X, HUANG Z X, WANG Y L, et al. A dynamic evolution model of disequilibrium network traffic flow with quantity regulation of congestion[J]. Journal of Traffic and Transportation Engineering, 2018, 18(3): 167-179. [4] DE MORAES RAMOS G, MAI T E, DAAMEN W, et al. Route choice behaviour and travel information in a congested network: static and dynamic recursive models[J]. Transportation Research Part C: Emerging Technologies, 2020, 114: 681-693. doi: 10.1016/j.trc.2020.02.014 [5] ZHU S J, LEVINSON D. Do people use the shortest path? An empirical test of Wardrop’s first principle[J]. PLoS One, 2015, 10(8): 0134322.1-0134322.22. [6] FAYYAZ M, BLIEMER M C J, BECK M J, et al. Stated choices and simulated experiences: differences in the value of travel time and reliability[J]. Transportation Research Part C: Emerging Technologies, 2021, 128: 103145.1-103145.19. [7] WATLING D. User equilibrium traffic network assignment with stochastic travel times and late arrival penalty[J]. European Journal of Operational Research, 2006, 175(3): 1539-1556. doi: 10.1016/j.ejor.2005.02.039 [8] ZHU Z, XIONG C F, CHEN X Q, et al. Integrating mesoscopic dynamic traffic assignment with agent-based travel behavior models for cumulative land development impact analysis[J]. Transportation Research Part C: Emerging Technologies, 2018, 93: 446-462. doi: 10.1016/j.trc.2018.06.011 [9] ZHANG Y F, KHANI A. An algorithm for reliable shortest path problem with travel time correlations[J]. Transportation Research Part B: Methodological, 2019, 121: 92-113. doi: 10.1016/j.trb.2018.12.011 [10] XU X D, CHEN A, ZHOU Z, et al. A multi-class mean-excess traffic equilibrium model with elastic demand[J]. Journal of Advanced Transportation, 2014, 48(3): 203-222. doi: 10.1002/atr.205 [11] SUN C, CHENG L, ZHU S L, et al. Multiclass stochastic user equilibrium model with elastic demand[J]. Transportation Research Record: Journal of the Transportation Research Board, 2015, 2497(1): 1-11. doi: 10.3141/2497-01 [12] DI X, LIU H X. Boundedly rational route choice behavior: a review of models and methodologies[J]. Transportation Research Part B: Methodological, 2016, 85: 142-179. doi: 10.1016/j.trb.2016.01.002 [13] 赵传林,黄海军. 基于满意准则的有限理性用户均衡流量分配性质研究[J]. 系统工程理论与实践,2014,34(12): 3073-3078. doi: 10.12011/1000-6788(2014)12-3073ZHAO Chuanlin, HUANG Haijun. Properties of boundedly rational user equilibrium under satisficing rule in traffic assignment problem[J]. Systems Engineering—Theory & Practice, 2014, 34(12): 3073-3078. doi: 10.12011/1000-6788(2014)12-3073 [14] 张新洁,关宏志,赵磊,等. 有限理性视野下出行者出行方式选择分层Logit模型研究[J]. 交通运输系统工程与信息,2018,18(6): 110-116. doi: 10.16097/j.cnki.1009-6744.2018.06.016ZHANG Xinjie, GUAN Hongzhi, ZHAO Lei, et al. Nested logit model on travel mode choice under boundebly rational view[J]. Journal of Transportation Systems Engineering and Information Technology, 2018, 18(6): 110-116. doi: 10.16097/j.cnki.1009-6744.2018.06.016 [15] GONZÁLEZ RAMÍREZ H, LECLERCQ L, CHIABAUT N, et al. Travel time and bounded rationality in travellers’ route choice behaviour: a computer route choice experiment[J]. Travel Behaviour and Society, 2021, 22: 59-83. doi: 10.1016/j.tbs.2020.06.011 [16] SUN C, LI M H, CHENG L, et al. Boundedly rational user equilibrium with restricted unused routes[J]. Discrete Dynamics in Nature and Society, 2016, 2016: 9848916.1-9848916.11. [17] WANG D, LIAO F X, GAO Z Y, et al. Tolerance-based strategies for extending the column generation algorithm to the bounded rational dynamic user equilibrium problem[J]. Transportation Research Part B: Methodological, 2019, 119: 102-121. doi: 10.1016/j.trb.2018.11.008 [18] YU H, ZHU S L, YANG J, et al. A Bayesian method for dynamic origin−destination demand estimation synthesizing multiple sources of data[J]. Sensors, 2021, 21(15): 4971.1-4971.20. [19] LEBLANC L J. Mathematical programming algorithms for large scale network equilibrium and network design problems[D]. Evanston: Northwestern University, 1973 期刊类型引用(2)
1. 赵雪亭,胡立伟. 城市交通拥塞源参数反演性能综合对比. 西南交通大学学报. 2025(01): 243-252 .  本站查看
本站查看2. 毕瑞,吴红艳,孙婷,孟凡宁,孙玉刚. 临床药师参与临床决策的有限理性分析. 医学与哲学. 2023(03): 59-61 .  百度学术
百度学术其他类型引用(5)
-





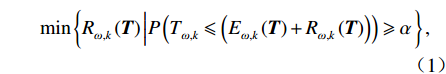
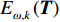

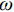
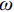
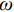
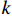
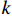
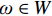
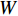
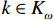
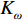
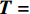
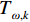
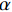
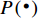
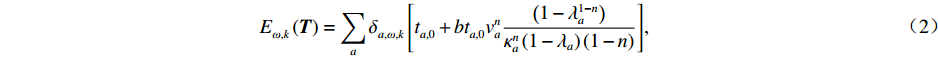

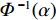
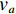
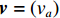
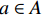
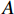
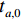
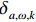
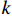
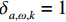
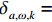
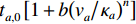
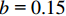

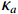
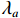
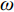
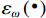

 下载:
下载:
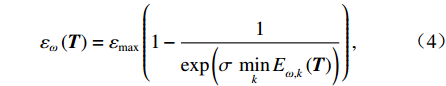

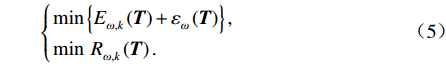
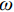
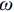
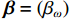
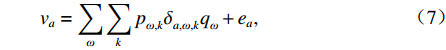
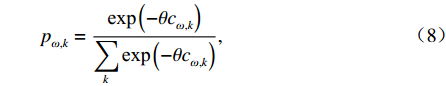
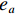

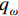
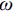
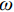
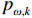
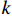
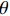
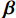
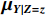
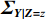
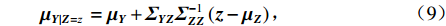
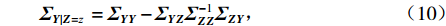
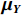

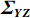
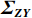


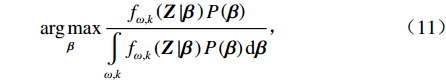
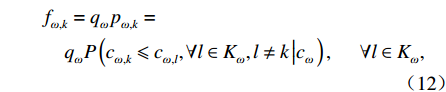
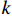
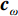
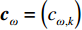
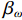
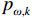
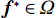
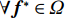

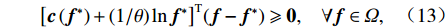
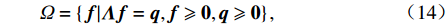
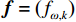
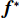
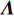
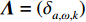
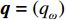
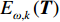
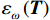
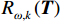
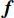
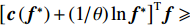
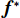
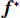
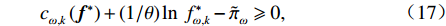

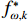

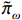
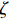
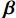
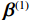
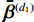
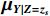
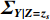
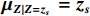

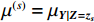
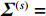
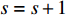

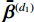
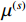
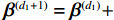
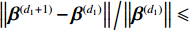
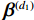
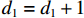
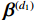
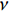
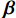
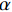
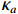
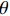

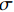
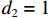
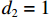
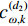
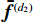
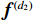
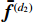
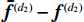
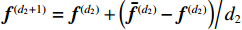
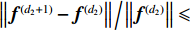
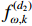
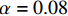
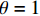


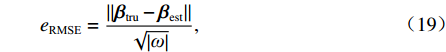
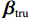
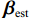
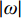
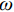
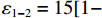
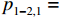
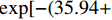
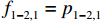



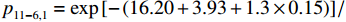
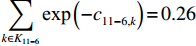
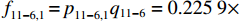


 下载:
下载:
 百度学术
百度学术