Model-Free Adaptive Control for Single-Degree-of-Freedom Magnetically Levitated System
-
摘要:
针对单自由度磁悬浮系统的非线性及难以建立精确数学模型的问题,将全格式无模型自适应控制(FFDL-MFAC)方法应用于单自由度磁悬浮系统,首先,采用无模型自适应控制算法、伪梯度估计算法、伪梯度重置算法和单自由度磁悬浮系统的动态化数据模型,设计单自由度磁悬浮系统的控制器;然后,仿真分析MFAC控制参数对单自由度磁悬浮系统控制效果的影响及对阶跃响应信号、干扰信号和噪声信号的响应特性;最后,在磁悬浮球实验装置上进行实验验证. 研究结果表明:全格式无模型自适应控制方法只需采集单自由度磁悬浮系统在工作状态下的I/O数据,无需建立单自由度磁悬浮系统精确数学模型,通过设定全格式无模型自适应控制器参数即可使控制器具备良好的自适应性和鲁棒性,实现高精度稳定悬浮控制;与PID相比,FFDL-MFAC将系统的超调量降低了0.005,稳定悬浮位移的误差均方根减小了0.2607.
Abstract:Aiming at the problem of nonlinear and difficult to establish accurate mathematical model of a single-degree-of-freedom magnetically levitated system, the model-free adaptive control method based on full-format dynamic linearization (FFDL-MFAC) was applied to a single-degree-of-freedom magnetically levitated system. Firstly, model-free adaptive control algorithm, pseudo gradient estimation algorithm, pseudo gradient reset algorithm and dynamic data model of single degree of freedom magnetic levitation system were used to design the controller of single degree of freedom magnetic levitation system. Then the influence of MFAC control parameters on the control effect of the single-degree-of-freedom magnetically levitated system and the response characteristics of step response signal, interference signal and noise signal are analyzed by simulation, and the experimental verification was carried out on the magnetic levitation ball experimental device. Finally, the experimental verification was carried out on the magnetic levitation ball experimental device. The results show that the FFDL-MFAC method only needs to collect the I/O data of the single-degree-of-freedom magnetically levitated system under the working state, and does not need to establish an accurate mathematical model of the single-degree-of-freedom magnetically levitated system. The high precision and stable suspension control can be realized by setting the parameters of the model free adaptive controller, and the controller has good adaptability and robustness. Compared with PID, FFDL-MFAC reduces the overshoot of the system by 0.005, and the root mean square error of stable suspension displacement is reduced by 0.2607.
-
Key words:
- magnetic levitation /
- adaptive algorithms /
- model-free adaptive control /
- pseudo-gradient
-
磁悬浮技术已广泛应用于航空航天、医疗设备、能量回收、透平机械等领域[1-4]. 对于单自由度磁悬浮系统而言,其控制精度的高低将直接影响系统的稳定性[5],因此如何提高控制精度一直是磁悬浮技术研究的重点.
目前,针对磁悬浮技术的控制问题,已有许多学者进行研究,并取得了丰富的成果[6-9]. 吕治国等[10]通过状态反馈法建立精确线性化磁悬浮小球的数学模型,并采用极点配置法与在线参数辨识相结合的方法实现了单自由度磁悬浮系统的自适应控制. 朱坚民等[11]通过在线训练神经网络辨识器,建立单自由度磁悬浮系统误差与控制量的动态模型,在PID控制器基础上进一步进行反馈补偿,该控制方法与PID控制器相比,其控制精度有了很明显的增加. 张井岗等[12]将分数阶PDμ(对PD控制中的微分项进行μ阶求导)作为滑模面函数进行滑模控制,有效解决了单自由度磁悬浮系统在传统滑模控制中的抖振现象. 宋荣荣等[13]通过模糊PID原理构造单自由度磁悬浮系统的数学模型,制定相关模糊控制规则,并设计出一种可以在线调整PID参数的控制器,减小了系统的跟踪误差. 为避免高速磁悬浮控制过程由于重力使转子发生小角度的公转,夏长峰等[14]提出一种PID与滤波交叉反馈的方式,通过DSP (digital signal processor)和FPGA (field programmable gate array)搭建数字控制系统,实验结果表明,这种方法可以很好地避免转子发生小角度的进动.
以上控制算法都是通过建立精确的模型来实现单自由度磁悬浮系统的控制,模型越精确,控制效果越好. 然而,由于单自由度磁悬浮系统漏磁、磁路程耦合等因素的存在,精确的磁悬浮模型通常难以获得. 因此,针对被控对象数字模型难以建立的问题,有学者提出了数据驱动控制的方法,如迭代反馈控制[15]、迭代学习控制[16]、虚拟参考反馈控制[17-18]和无模型自适应控制(model-free adaptive contorl, MFAC)[19-20]等. 在众多的数据驱动方法中,较为典型的是PID和MFAC. 但PID自适应差、鲁棒性不强,所以不适合用于具有强非线性的系统中. 与PID相比,MFAC不仅鲁棒性强、自适应性好、概念简单易懂而且算法的稳定性与收敛性均具有严格的数学证明[21-25],因此,在许多实际系统中得到了广泛的应用,如地面车辆[26]、气压制冷系统[27]、广域电力系统[28]等,但MFAC在磁悬浮领域的应用较少,文献[29]提出了一种单自由度磁悬浮系统的无模型自适应控制方法,仿真分析了MFAC与PID两种控制方法对磁悬浮球的控制效果,结果表明该方法对单自由度磁悬浮系统的控制是有效的.
本文将基于全格式动态线性化的无模型自适应控制方法[29]应用于单自由度磁悬浮系统,设计单自由度磁悬浮系统无模型自适应控制器,仿真分析了MFAC控制参数对单自由度磁悬浮系统控制效果的影响及对阶跃响应信号、干扰信号和噪声信号的响应特性,最后在磁悬浮球实验台上进行实验验证. 仿真及实验结果表明:全格式无模型自适应控制方法能够最大限度地摆脱单自由度磁悬浮系统对精确模型的依赖,优化系统动态性能,且基于跟踪的全格式无模型自适应控制器在稳定时间及系统的动态性能等方面明显优于PID控制器.
1. 单自由度磁悬浮系统
多自由度磁悬浮系统在满足一定假设条件下,可以等效为多个独立的单自由度系统. 因此,以单自由度磁悬浮系统作为被控对象,分析MFAC对单自由度磁悬浮系统的控制效果具有很好的指导作用.
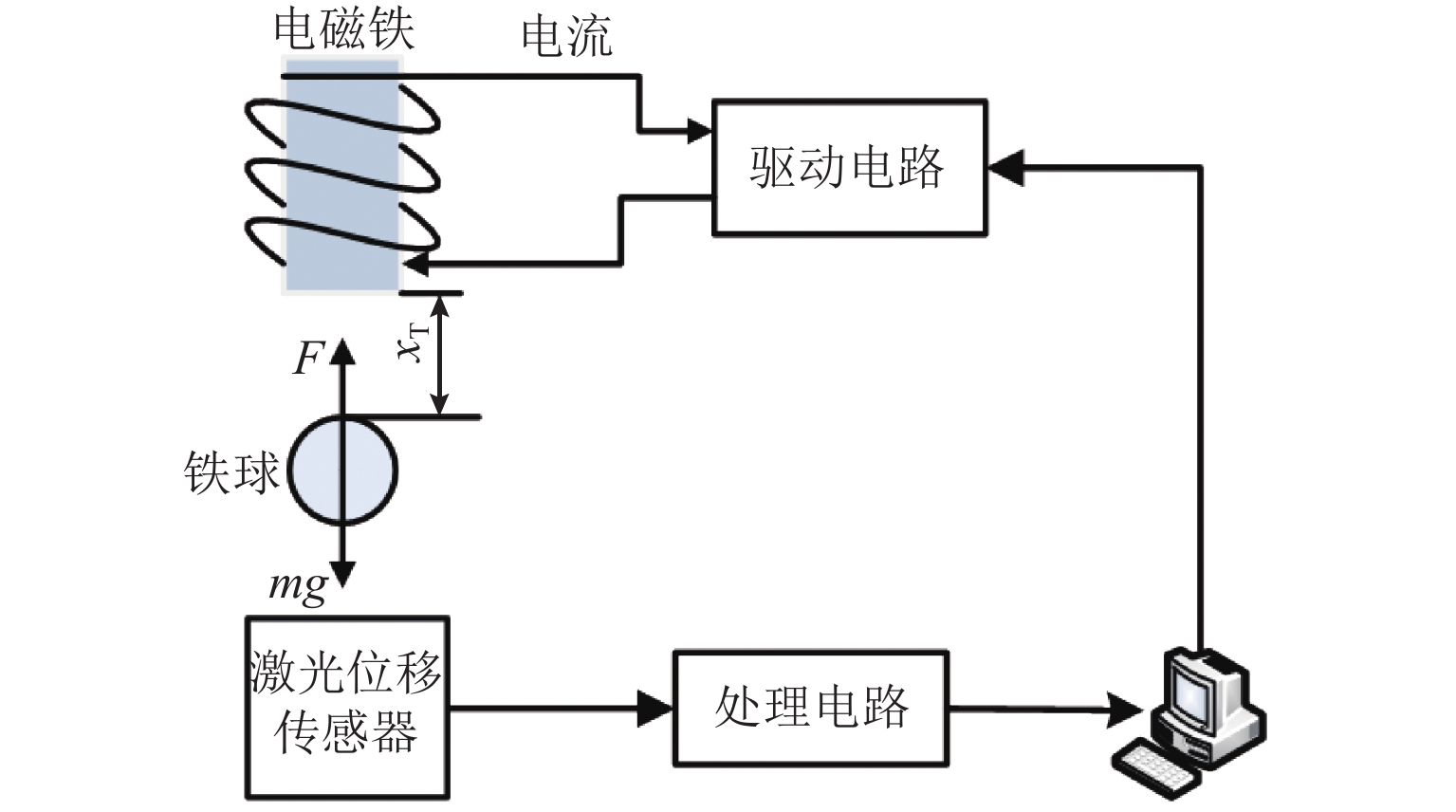
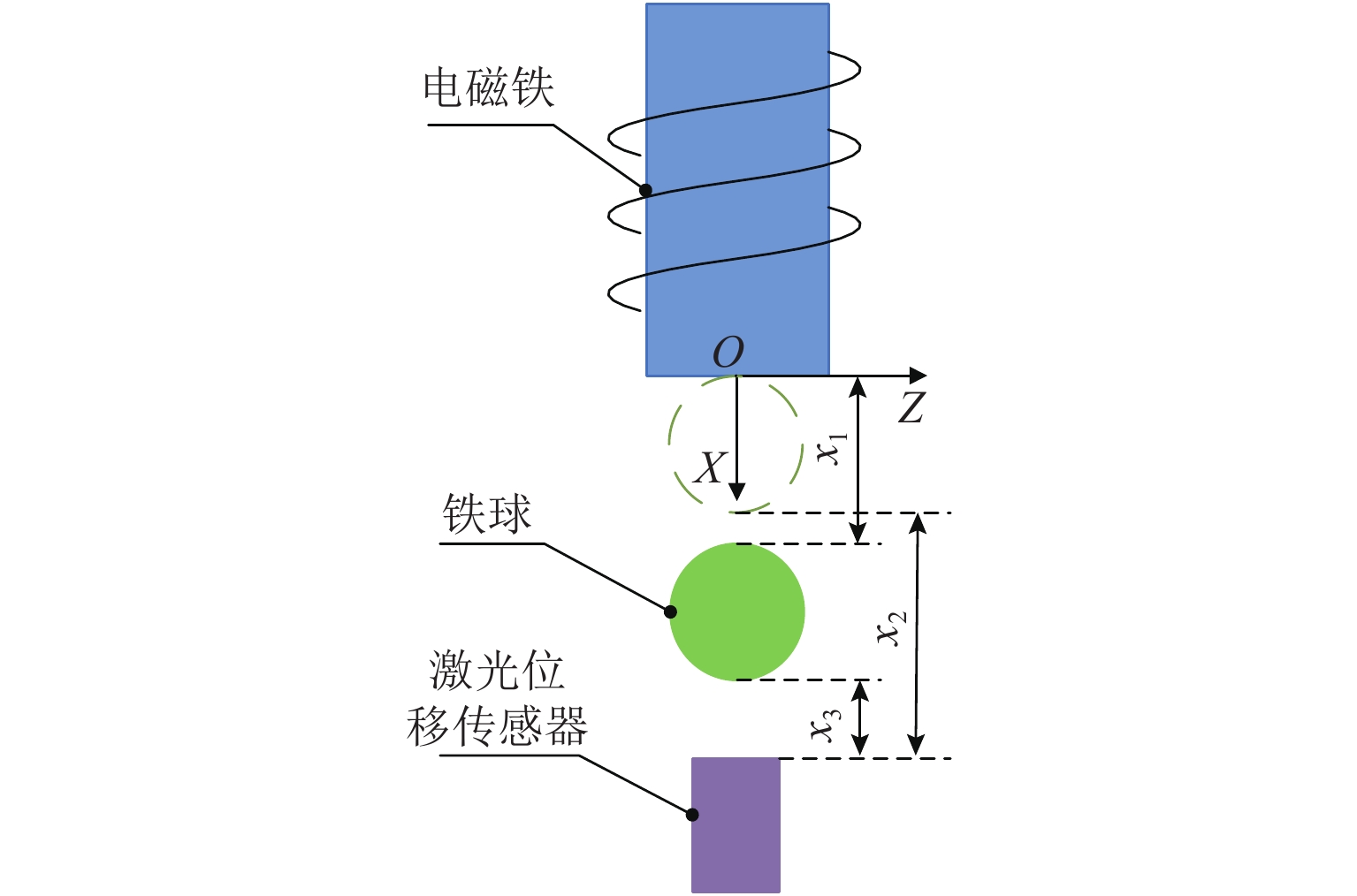
单自由度磁悬浮系统如图1所示,主要由电磁铁、铁球、激光位移传感器、驱动电路和控制器组成,图中:m为铁球的质量;g为重力加速度;F为电磁力;xT为铁球的位移. 当电磁铁产生的电磁力与铁球的重力相等时,铁球即实现悬浮.
1.1 单自由度磁悬浮系统数学模型
为简化计算,对单自由度磁悬浮系统做出如下假设[29]:
假设1 每匝线圈中通过的磁通量相同.
假设2 功率放大器不存在时间滞后.
则由图1可以推导出单自由度磁悬浮系统的动力学方程为
{md2xdt2=F(ic,x)+mg,F(ic,x)=−μ0AN24(icx)2,mg+F(i0,x0)=0, (1) 式中:x为铁球质心到电磁铁磁极表面的瞬时气隙;ic为电磁铁中瞬时电流;x0为铁球的平衡气隙;i0为电磁铁中的平衡电流;F(ic, x)为电磁吸力;μ0为空气磁导率;A为铁芯的导磁截面积;N为电磁铁线圈匝数;F(i0, x0)为平衡位置(i0,x0)处电磁铁对小球的电磁吸力.
将式(1)在平衡位置(i0,x0)处进行泰勒级数展开,可得
F(ic,x)=F(i0,x0)+2Ki0x20(ic−i0)−2Ki20x30(x−x0), (2) 式中:
K=−μ0AN2/4. 将式(2)代入式(1)可得单自由度磁悬浮系统的数学模型为
md2xdt2=2Ki0x20(ic−i0)−2Ki20x30(x−x0). (3) 对式(3)进行拉普拉斯变化,并代入式(1)中,可得单自由度磁悬浮系统的开环传递函数为
G0(s)=CAs2−B, (4) 式中:C = −1/Ka,Ka为功率放大器的增益;s为复数;A=i0/(2g);B=i0/x0.
单自由度磁悬浮系统实验台的物理参数如表1所示.
表 1 单自由度磁悬浮系统的物理参数Table 1. Physical parameters of a single-degree-of-freedom magnetically levitated system符号 参数 值 m 小球质量/g 94 Ka 放大系数 6.508 A 系数 0.02012 B 系数/(A·m−1) 39.433 将表1中的物理量值代入式(4),得到单自由度磁悬浮系统的开环传递函数为
G0(s)=7.6367s2−1960. (5) 为确保不失真的恢复模拟信号,根据香农采样定理,确定采样时间T=0.001 s,对式(5)进行离散化后可知单自由度磁悬浮系统的动态数学模型为
y(k+1)=2.002y(k)+y(k−1)+0.000003819u(k)+0.000003819u(k−1), (6) 式中:k为采样时刻,k=1,2,
⋯ ,N,N为最大采样时刻;u(k) 和u(k−1)分别为时刻k和时刻k−1系统的输入值;y(k)、y(k−1)和y(k + 1)分别为时刻k、时刻k−1和时刻k + 1系统的输出值.2. 全格式无模型自适应控制器设计
2.1 单自由度磁悬浮系统的动态线性化
定义
HLy,Lu(k)∈RLy,Lu 为在区间[k−Lu+1,k] 内的所有控制输入信号以及在区间[k−Ly+1,k] 内所有输出信号组成的向量,即HLy,Lu(k)=[y(k)⋯y(k−Ly+1)u(k)⋯u(k−Lu+1)]T. 当满足k≤0时,
HLy,Lu(k)=0Ly+Lu ,其中Ly,Lu(0⩽ 称为系统的伪阶数, ny和nu为系统的阶数.对于单自由度磁悬浮系统(6),满足如下假设:
假设1 单自由度磁悬浮系统(6)输出可控可测.
假设2 除有限的时刻点,
f({\text{•}} ) 关于第(ny + 2)个变量的偏导数是连续的[25].假设3 除有限的时刻点,单自由度磁悬浮系统(6)满足广义Lipschitz条件,即任意采样时刻
{k}_{1}\ne {k}_{2},{k}_{1},{k}_{2}\geqslant 0, 和{{{\boldsymbol{H}}}}_{{{L}}_{\mathrm{y},}{{L}}_{\mathrm{u}}}\left({k}_{1}\right)\ne {{{\boldsymbol{H}}}}_{{{L}}_{\mathrm{y},}{{L}}_{\mathrm{u}}}\left({k}_{2}\right) ,有| {y({k_1} + 1) - y({k_2} + 1)} | \leqslant b\| {{{{{\boldsymbol{H}}}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}({k_1}) - {{{{\boldsymbol{H}}}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}({k_2})} \| , 式中:
y({k}_{j} + 1)=f(y({k}_{j}),\cdots ,y({k}_{j}-{n}_{{\rm{y}}}),u({k}_{j}),\cdots ,u({k}_{j}- {n}_{{\rm{u}}})) ,j = 1,2 ;b为大于0的常数.因此,根据引理[25]可知,给定
0\leqslant {L}_{{\rm{y}}}\leqslant {n}_{y},1\leqslant {L}_{{\rm{u}}}\leqslant {n}_{{\rm{u}}} ,当{{{\boldsymbol{H}}}}_{{{L}}_{\rm{y},}{{L}}_{\rm{u}}}\left(k\right)-{{{\boldsymbol{H}}}}_{{{L}}_{\rm{y},}{{L}}_{\rm{u}}}\left(k-1\right)\ne 0 时,存在一个称为伪梯度的时变参数向量{\boldsymbol{\varPhi }}_{{{\rm{f}}},{{L}}_{\rm{y},}{{L}}_{\rm{u}}}\left(k\right)\in {R}^{{{L}}_{\rm{y},}{{L}}_{\rm{u}}} ,使得单自由度磁悬浮系统可转变为式(7)的动态化数据模.y(k + 1) = y(k) + {{{\boldsymbol{\varPhi}} }}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}^{\rm{T}}(k)\Delta {{{{\boldsymbol{H}}}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k), (7) 式中:
\Delta {{{{\boldsymbol{H}}}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k) = {{{\boldsymbol{H}}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k) - {{{\boldsymbol{H}}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k - 1) ;{{\boldsymbol{\varPhi }}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k) = \left[ {{\varPhi _1}(k)\cdots {\varPhi _{{{{L}}_{\rm{y}}}}}(k)} \right. {\left. {{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)\cdots {\varPhi _{{{{L}}_{\rm{y}}} + {{{L}}_{\rm{u}}}}}(k)} \right]^{\text{T}}} .Φj(k)为
{\boldsymbol{\varPhi }}_{{{\rm{f}}},{{L}}_{\mathrm{y},}{{L}}_{\mathrm{u}}}\left(k\right) 中第j项的数值,j=1,\cdots , Ly + Lu. 式(7)是单自由度磁悬浮系统(6)的一种等价的动态线性化表示,是用于设计控制器的线性时变数据模型.2.2 单自由度磁悬浮系统的MFAC控制算法
对式(7)采用式(8)所示的函数作为控制输入准则函数.
\begin{split} &J(u (k)) = {| {{y^*}(k + 1) - y(k + 1)} |^2} +\\ &\quad \lambda {| {u(k) - u(k - 1)} |^2} , \end{split} (8) 式中:
\lambda > 0 为控制算法的权重因子,用来控制输入量变化;y*(k + 1)为期望的输出信号.将式(7)代入式(8)中,对
u\left(k\right) 求导,并令其等于0,可得到式(9)所示的控制算法.u(k) = \left\{ \begin{array}{l} u(k - 1) + \dfrac{{{\rho _{{{{L}}_{\rm{y}}} + 1}}{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)(({y^*}(k + 1) - y(k)))}}{{\lambda + {{\left| {{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)} \right|}^2}}} - \dfrac{{{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)\displaystyle\mathop \sum \limits_{j = 1}^{{{{L}}_{\rm{y}}}} {\rho _j}{\varPhi _j}(k)\Delta y(k - j + 1)}}{{\lambda + {{\left| {{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)} \right|}^2}}},\;\quad{L_{\rm{u}}} = 1, \hfill \\ u(k - 1) + \dfrac{{{\rho _{{{{L}}_{\rm{y}}} + 1}}{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)(({y^*}(k + 1) - y(k)))}}{{\lambda + {{\left| {{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)} \right|}^2}}} - \dfrac{{{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)\displaystyle\mathop \sum \limits_{j = 1}^{{L_{\rm{y}}}} {\rho _j}{\varPhi _j}(k)\Delta y(k - j + 1)}}{{\lambda + {{\left| {{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)} \right|}^2}}}- \hfill \\ \quad \dfrac{{{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)\displaystyle\mathop \sum \limits_{j = {L_{\rm{y}}} + 2}^{{L_{\rm{y}}} + {L_{\rm{u}}}} {\rho _j}{\varPhi _j}(k)\Delta u(k - {{{L}}_{\rm{y}}} - j + 1)}}{{\lambda + {{\left| {{\varPhi _{{{{L}}_{\rm{y}}} + 1}}(k)} \right|}^2}}},\;\quad{{{L}}_{\rm{u}}} \geqslant 2, \\ \end{array} \right. (9) 式中:加入控制算法的步长因子
{\rho }_{j}\in \left(0,1\right] ,是为了使控制算法更具有一般性; Δy(k − j + 1)= y(k − j + 1)− y(k − j);Δx(k − Ly − j + 1)= x(k − Ly − j + 1)− x(k − Ly − j).2.3 伪梯度估计算法
由式(9)可知,若要完成该控制算法,须知道伪梯度(PG)的值,但是由于单自由度磁悬浮系统的数学模型未知,伪梯度是一个时变参数,很难确定其真实值. 因此,采用如下PG估计准则函数对伪梯度进行估计:
\begin{split} &J({{\boldsymbol{\varPhi }} _{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k)) = \left[ {y(k) - y(k - 1) - } \right. \hfill \\&\quad {\left. {{\boldsymbol{\varPhi }}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}^{\rm{T}}(k)\Delta {{\boldsymbol{H}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k - 1)} \right]^2} + \hfill \\&\quad \mu {\left| {{{\boldsymbol{\varPhi }}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k) - {{{\boldsymbol{\hat \varPhi }}}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k - 1)} \right|^2} , \end{split} (10) 式中:μ>0,为伪梯度估计算法的权重因子;
{\hat{\boldsymbol{\varPhi }}}_{\mathrm{f},{{L}}_{\mathrm{y},}{{L}}_{\mathrm{u}}}\left(k\right) 为{\boldsymbol{\varPhi }}_{\mathrm{f},{{L}}_{\mathrm{y},}{{L}}_{\mathrm{u}}}\left(k\right) 的估计值,{{\boldsymbol{\hat \varPhi }}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k) = \left[ {{{\hat \varPhi }_1}(k)\;\cdots\;{{\hat \varPhi }_{{L_{\rm{y}}}}}(k)} \right.{\left. {{{\hat \varPhi }_{{{{L}}_{\rm{y}}} + 1}}(k)\;\cdots\;{{\hat \varPhi }_{{{{L}}_{\rm{y}}} + {{{L}}_{\rm{u}}}}}(k)} \right]^{\text{T}}}. 根据最优条件,对式(10)关于
{\boldsymbol{\varPhi }}_{f,{L}_{{\rm{y}},}{L}_{{\rm{u}}}}\left(k\right) 求极值,可得到PG的估计算法为\begin{split}& {{{\boldsymbol{\hat \varPhi }}}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k) = {{{\boldsymbol{\hat \varPhi }}}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k - 1) + \\& \frac{{\eta \Delta {{\boldsymbol{H}}_{{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}}(k - 1)(\Delta y(k) - {{{\boldsymbol{\hat \varPhi }}}^{\rm{T}}}_{{\rm{f}},{{{L}}_{\rm{y}}},{{{L}}_{\rm{u}}}}(k - 1)\Delta {{\boldsymbol{H}}_{{{L}_{\rm{y}}},{{L}_{\rm{u}}}}}(k - 1))}}{{\mu + {{\left\| {\Delta {{\boldsymbol{H}}_{{{L}_{\rm{y}}},{{L}_{\rm{u}}}}}(k - 1)} \right\|}^2}}}, \end{split} (11) 式中:
\eta \in \left(0,1\right],\mathrm{为}\mathrm{伪}\mathrm{梯}\mathrm{度}\mathrm{估}\mathrm{计}\mathrm{算}\mathrm{法}\mathrm{的} 步长因子,可以使该算法有更强的灵活性和一般性2.4 单自由度磁悬浮系统的控制器设计
针对本文所应用的单自由度磁悬浮系统,最终选取
{L}_{{\rm{y}}}=2,{L}_{{\rm{u}}}=1 ,为了使2.3中的伪梯度估计算法在单自由度磁悬浮系统中的位移跟踪能力更强,需要引入伪梯度重置算法:{\hat{{{\boldsymbol{\varPhi}} }}}_{\mathrm{f},2,1}\left(k\right)={\hat{{{\boldsymbol{\varPhi}} }}}_{\mathrm{f},\mathrm{2,1}}\left(1\right) ,如果\|{\hat{{{\boldsymbol{\varPhi}} }}}_{\mathrm{f},\mathrm{2,1}} \left(k\right)\|\leqslant \varepsilon 或\| \Delta {H}_{\mathrm{2,1}} ×(k-1) \| \leqslant \varepsilon 或{\rm{sign}}({\hat{{{\boldsymbol{\varPhi}} }}}_{\mathrm{f},\mathrm{2,1}}(k))\ne {\hat{{{\boldsymbol{\varPhi}} }}}_{\mathrm{f},\mathrm{2,1}}(1) . 其中:ε为一个充分小的正数;{\hat{\boldsymbol{\varPhi }}}_{\mathrm{f},\mathrm{2,1}}\left(1\right)\mathrm{为} {\hat{\boldsymbol{\varPhi }}}_{\mathrm{f},2,1}\left(k\right) 的初值.通过以上估计算法和重置算法可知,全格式无模型自适应控制方案为
\begin{split} &{{{\boldsymbol{\hat \varPhi }}}_{{\rm{f}},2,1}}(k) = {{{\boldsymbol{\hat \varPhi }}}_{{\rm{f}},2,1}}(k - 1) + \frac{{\eta \Delta {{\boldsymbol{H}}_{2,1}}(k - 1)}}{{\mu + \Delta {{\boldsymbol{H}}_{2,1}}{{(k - 1)}^2}}} \times \\&\quad (\Delta y(k) - {{{\boldsymbol{\hat \varPhi }}}_{{\rm{f}},2,1}}(k - 1)\Delta {{\boldsymbol{H}}_{2,1}}(k - 1)), \end{split} (12) \begin{split} &u(k) = u(k - 1) + \frac{{{\rho _3}{\varPhi _3}(k)(({y^*}(k + 1) - y(k)))}}{{\lambda + {{\left| {{\varPhi _3}(k)} \right|}^2}}} - \\&\quad \frac{{{\varPhi _3}(k)\left( {{\rho _1}{\varPhi _1}(k)\Delta y(k) + {\rho _2}{\varPhi _2}(k)\Delta y(k - 1)} \right)}}{{\lambda + {{\left| {{\varPhi _3}(k)} \right|}^2}}} . \end{split} (13) 3. 仿真分析
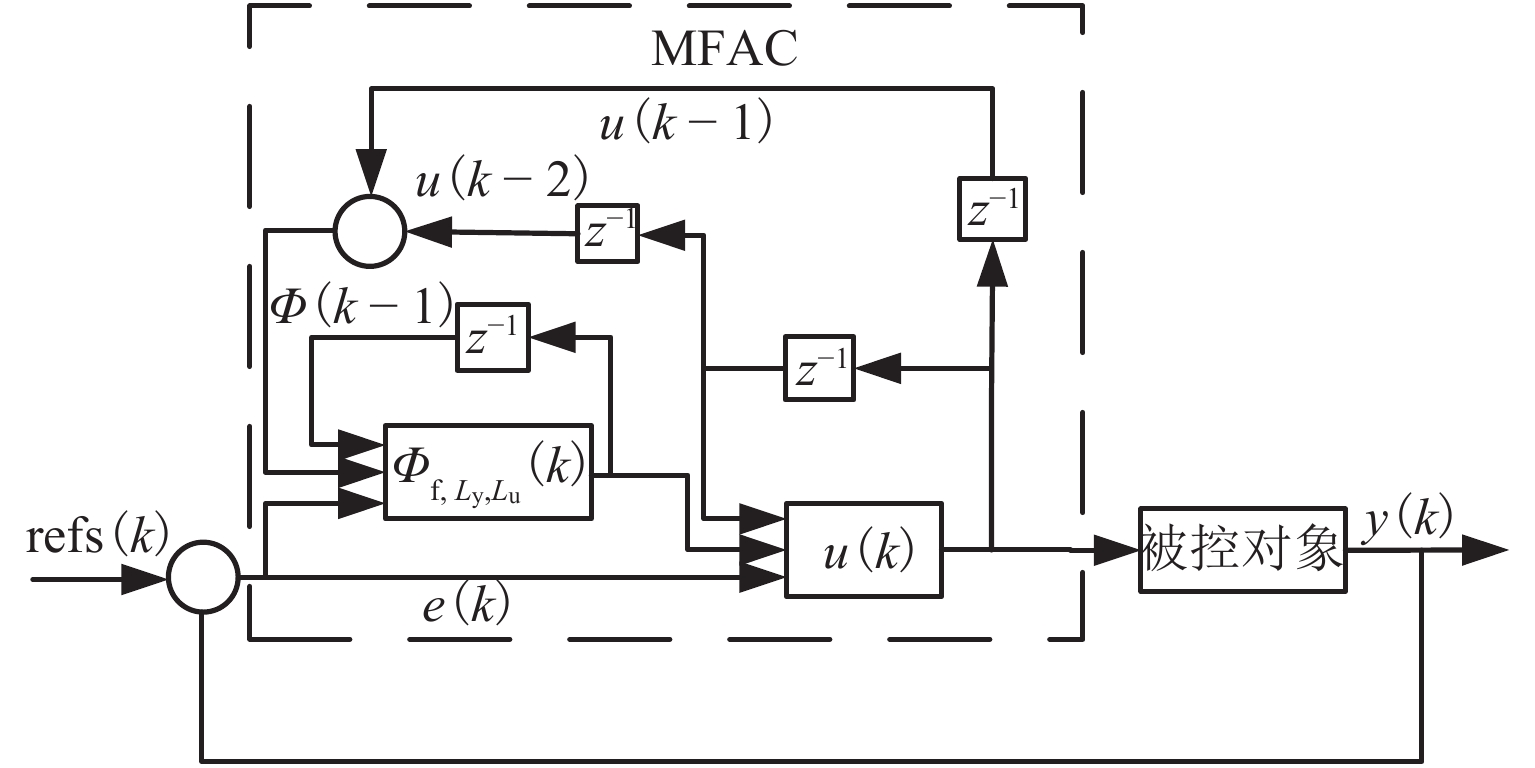
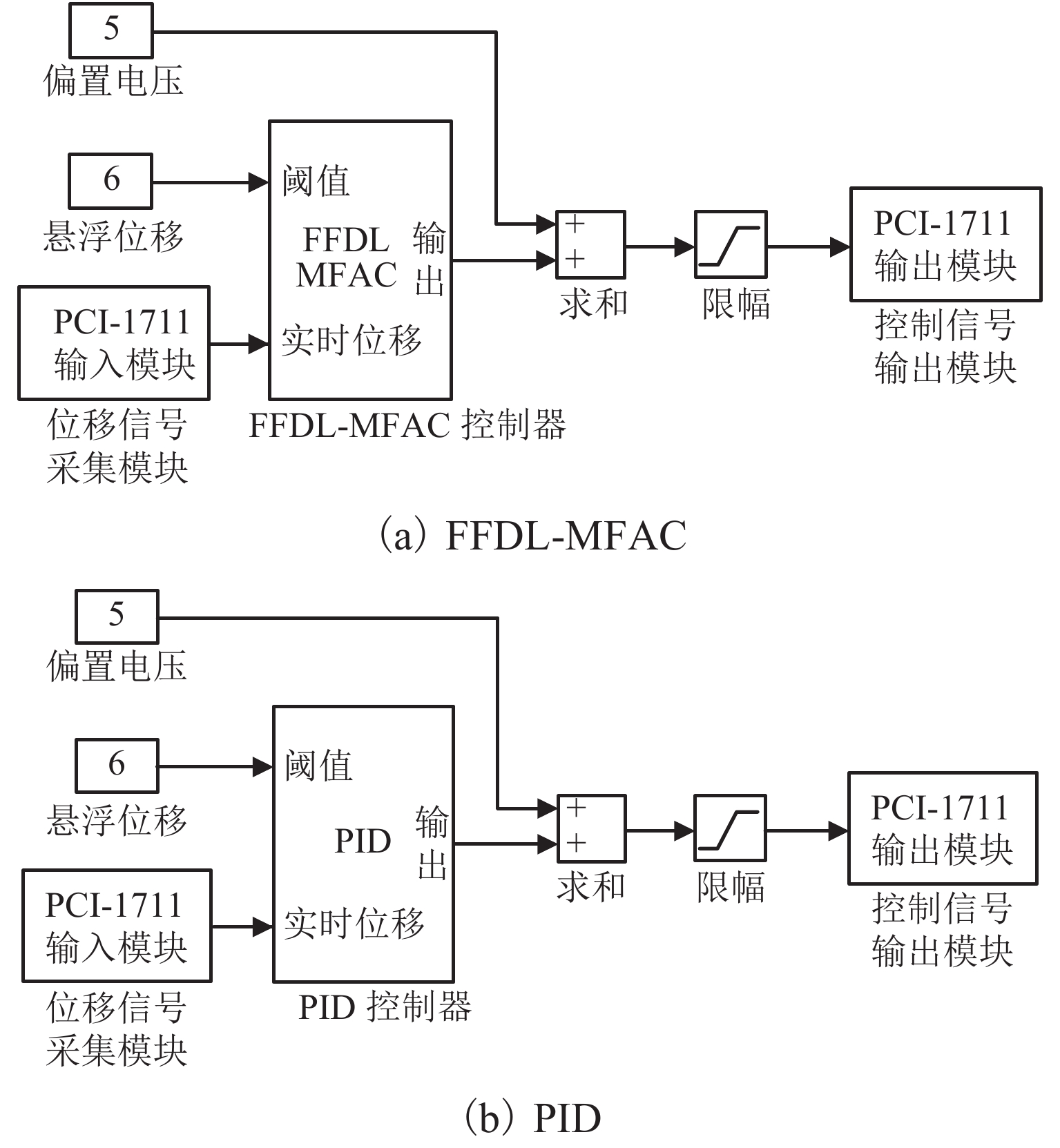
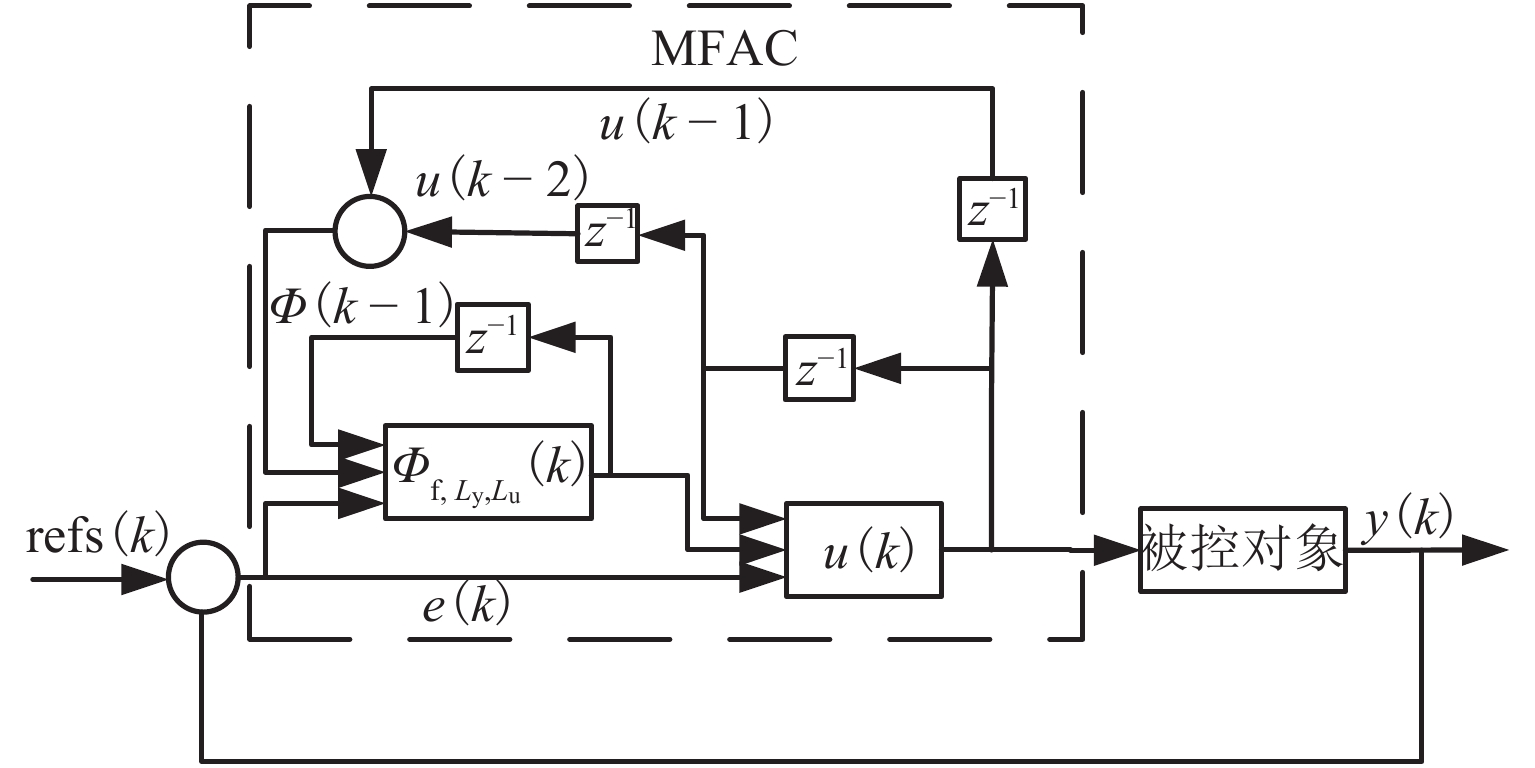
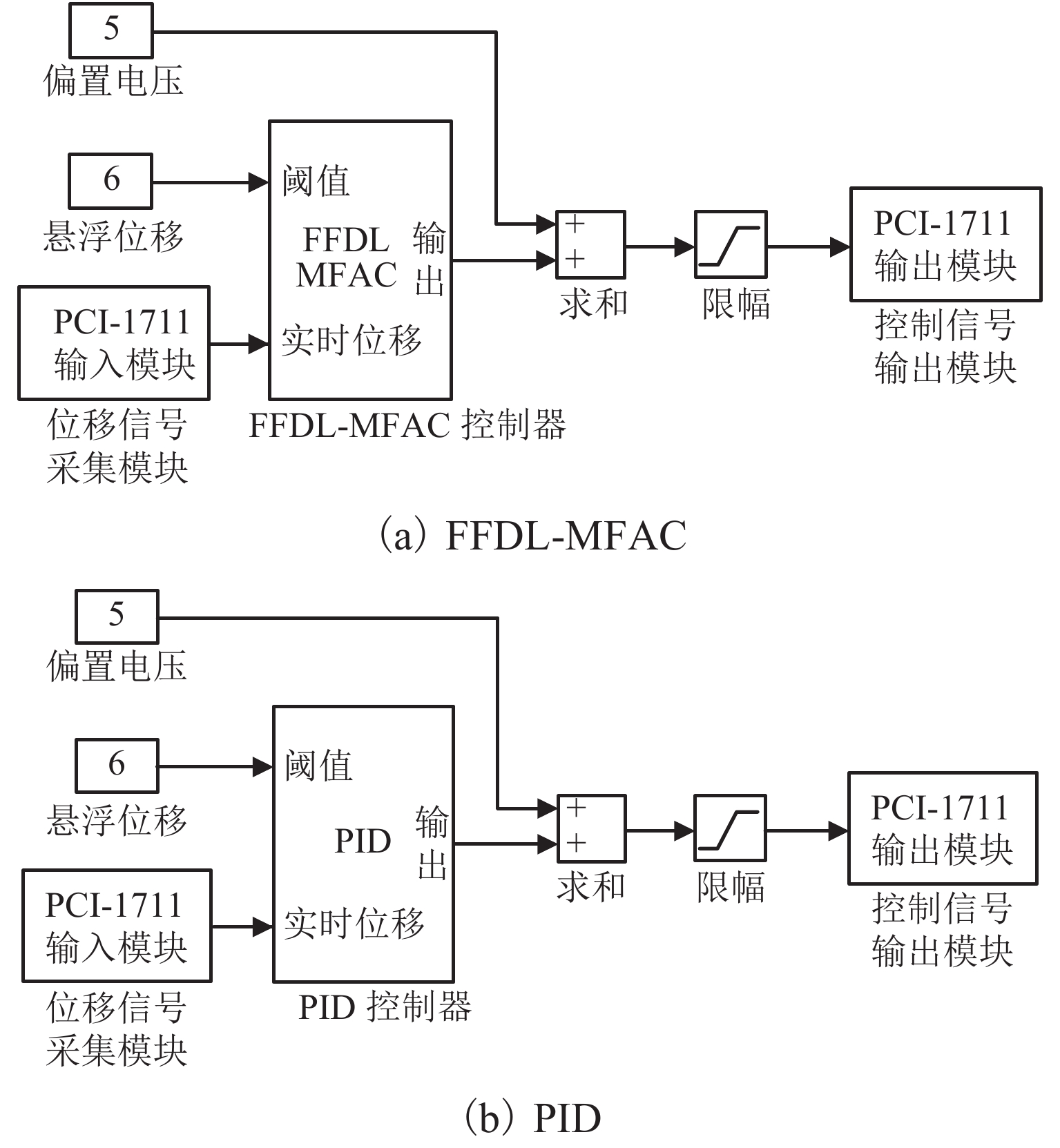
为验证上述分析的正确性,对FFDL-MFAC (model-free adaptive control method based on full-format dynamic linearization)控制器进行仿真分析,并以PID控制器作为对照组进行对比. FFDL-MFAC控制器如图2所示,PID控制器如式(14).
\left\{ \begin{array}{l} {\rm{d}}u(k) = {K_{\rm{p}}}(e(k) - e(k - 1)) + {K_{\rm{i}}}(e) + \\ \quad{K_{\rm{d}}}(e(k) - 2e(k - 1) + e(k - 2)),\\ u(k) = u(k - 1) + {\rm{d}}u(k), \end{array} \right. (14) 式中:e(k)为采样时刻k的误差;Kp、Ki、Kd分别为PID控制器中比例项、积分项、微分项的控制参数.
单自由度磁悬浮系统的参数如表1所示,两种算法的采样时间均为0.001 s. 为比较两种算法的控制性能,参考文献[30],采用误差均方根作为算法控制效果的评价指标,如式(15).
{{e}_{{\text{RMS}}}}{\text{ = }}\sqrt {\frac{1}{N}\sum\limits_{k = 1}^N {e{{(k)}^2}} } . (15) 在全格式无模型自适应控制方案中,步长因子和权重因子分别选取为ρ1=ρ2=0.6,ρ3=2.2,η=0.2,μ=0.1,λ=0.01,ε=0.1,伪梯度估计值的初始值设置为
{\widehat{\boldsymbol{\varPhi }}}_{\mathrm{f},2,1}\left(1\right)={\left[1\;000\;\quad 900\;\quad-0.1+0.1\mathrm{i}\right]}^{{\rm{T}}} . 在PID控制方案中,通过参数整定[31]得到最优PID控制参数为:Kp=2000,Ki=0.1,Kd=0.001.3.1 FFDL- MFAC自适应仿真分析
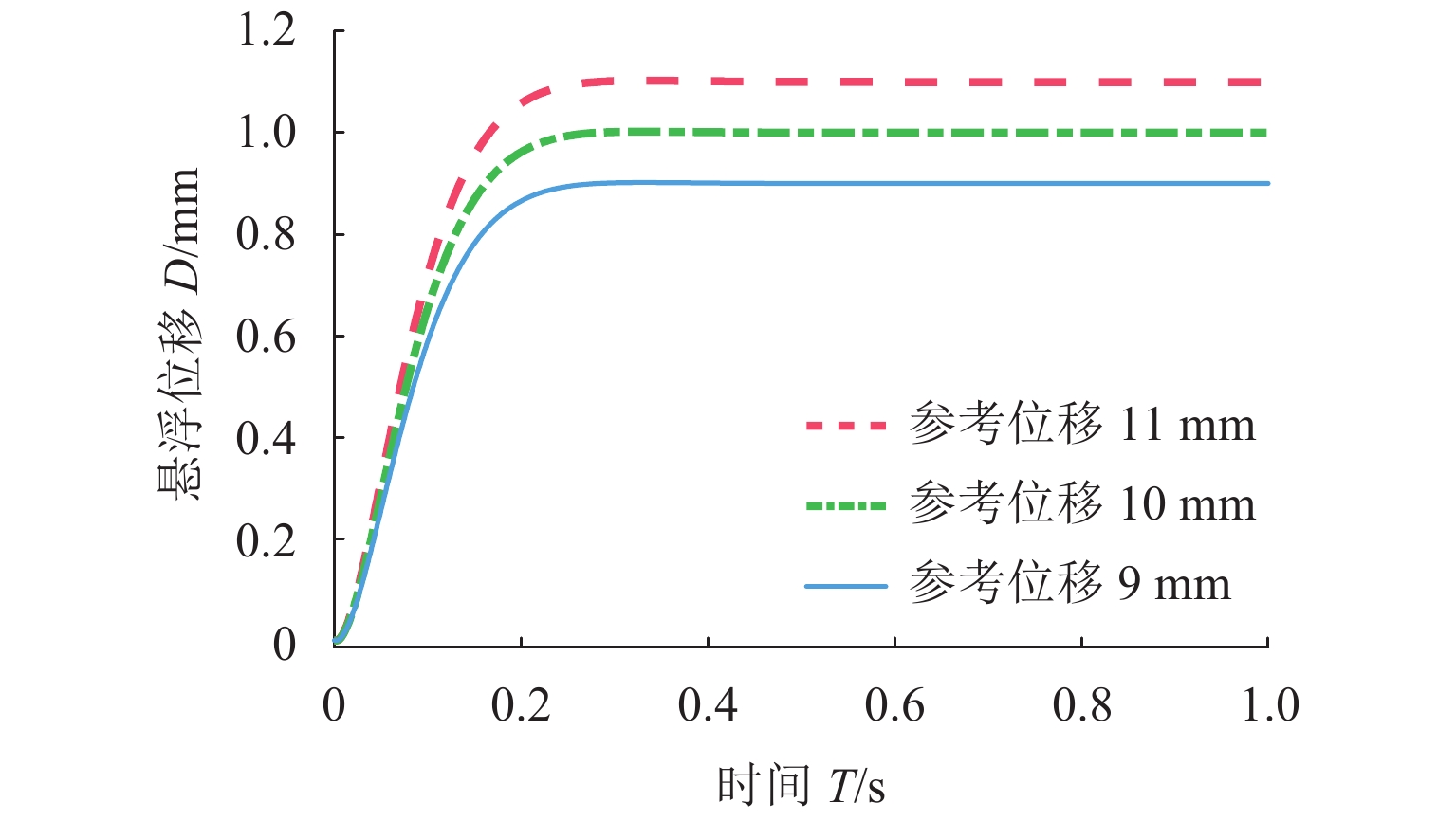
在控制参数不变的情况下,仅改变参考位移,则FFDL-MFAC的仿真结果如图3所示. 在不同的参考位移下,FFDL-MFAC控制器都可以跟踪期望信号较快地进入稳定状态.
3.2 FFDL- MFAC控制参数对悬浮效果的影响
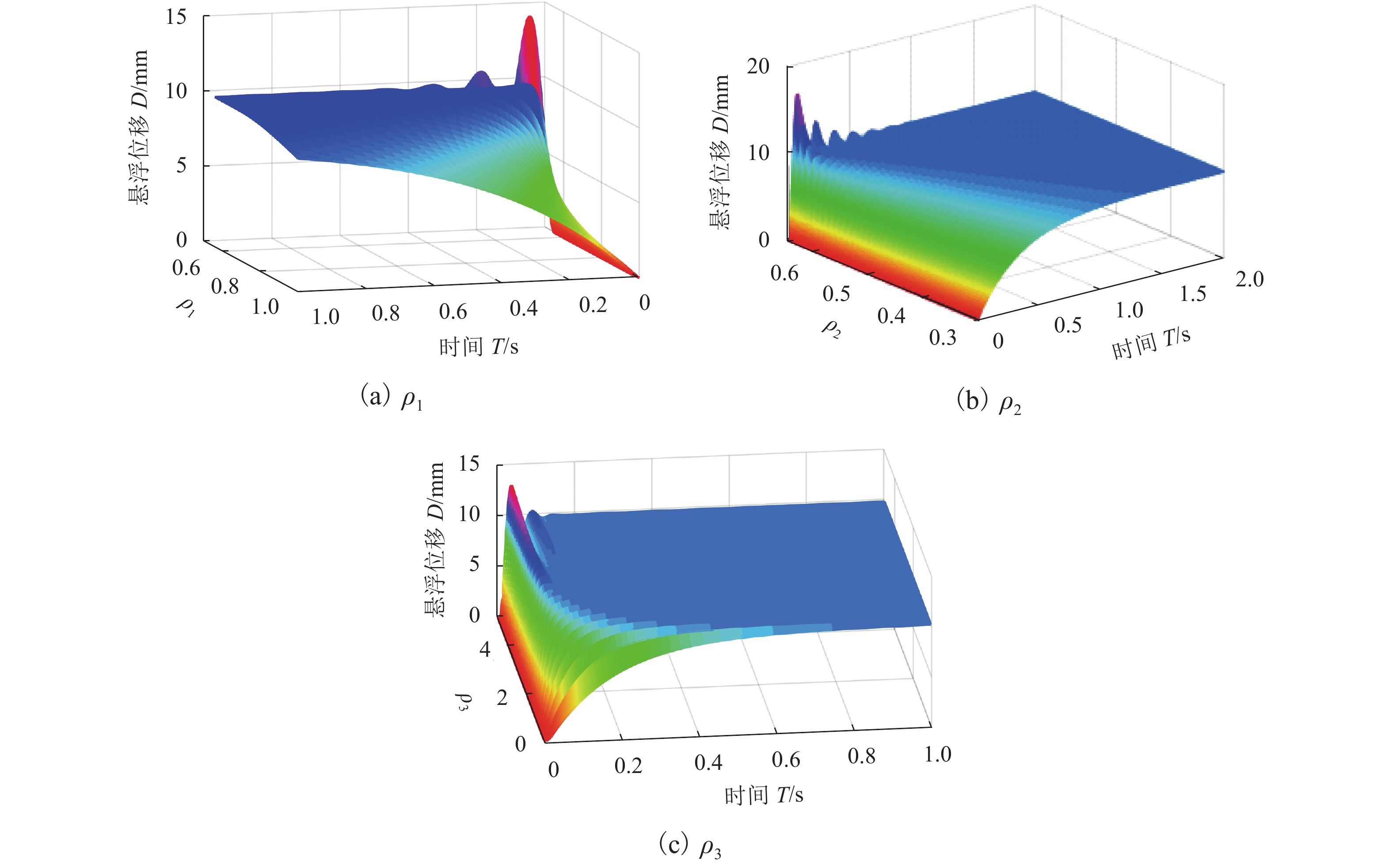
权重因子λ、步长因子ρ及伪梯度Φ的初始值是影响MFAC控制效果的主要参数. 为探清权重因子λ、ρ及伪梯度Φ的初始值对控制的影响,分别对其进行仿真分析.
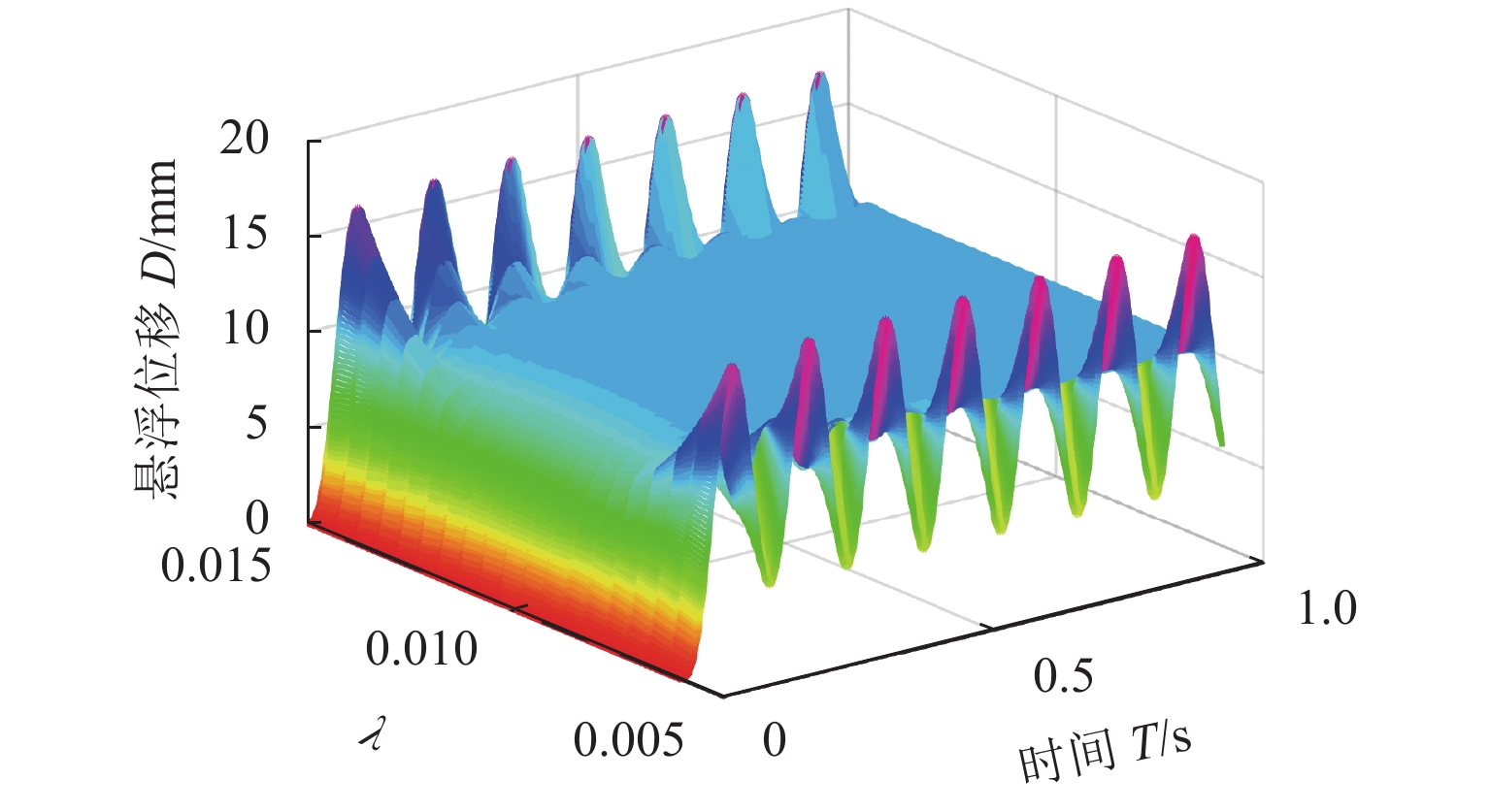
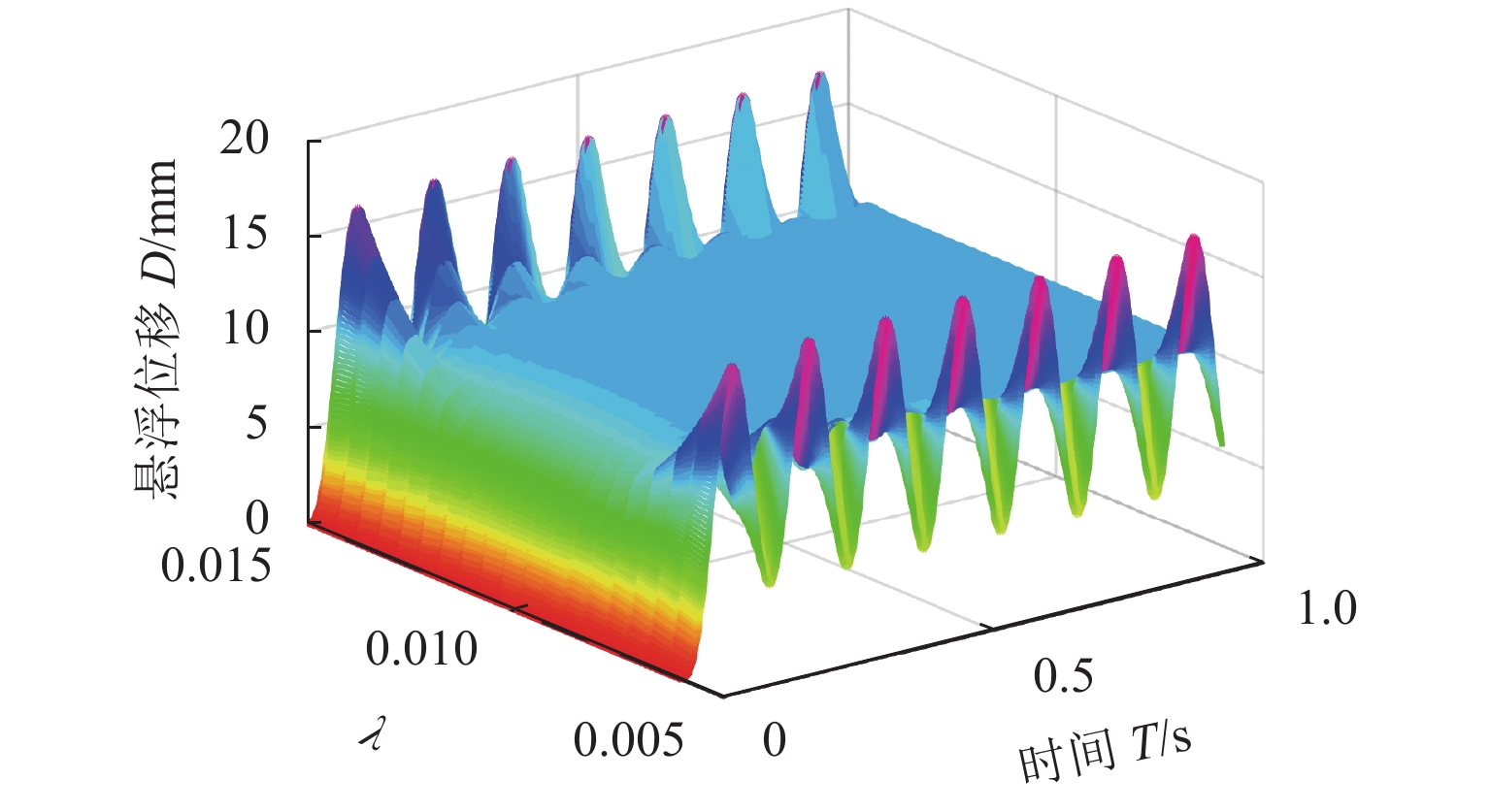
参数λ对悬浮效果的影响如图4所示,由图4可知:当λ在[ 0.0085, 0.0120]时,MFAC算法的响应速度基本不变,其稳定时间在0.18 s至0.20 s之间;当λ小于0.0085或大于0.0120时,MFAC算法的响应速度变快,但过快的响应速度会使得超调量增加.
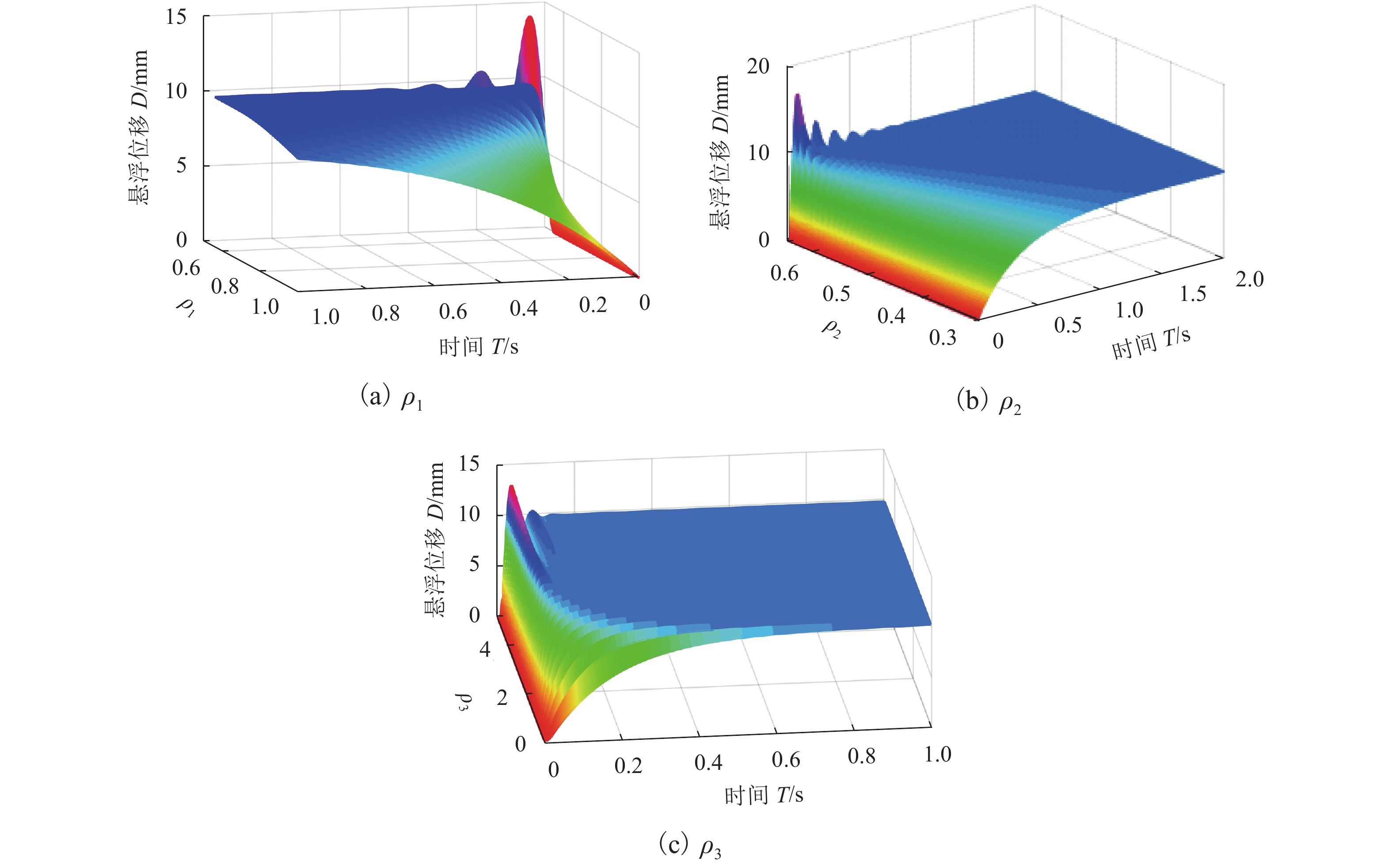
参数ρ对悬浮效果的影响如图5所示,MFAC算法的响应速度随ρ1取值的减小而增大,随ρ2、ρ3取值的增大而增大,但过快的响应速度会带来超调.
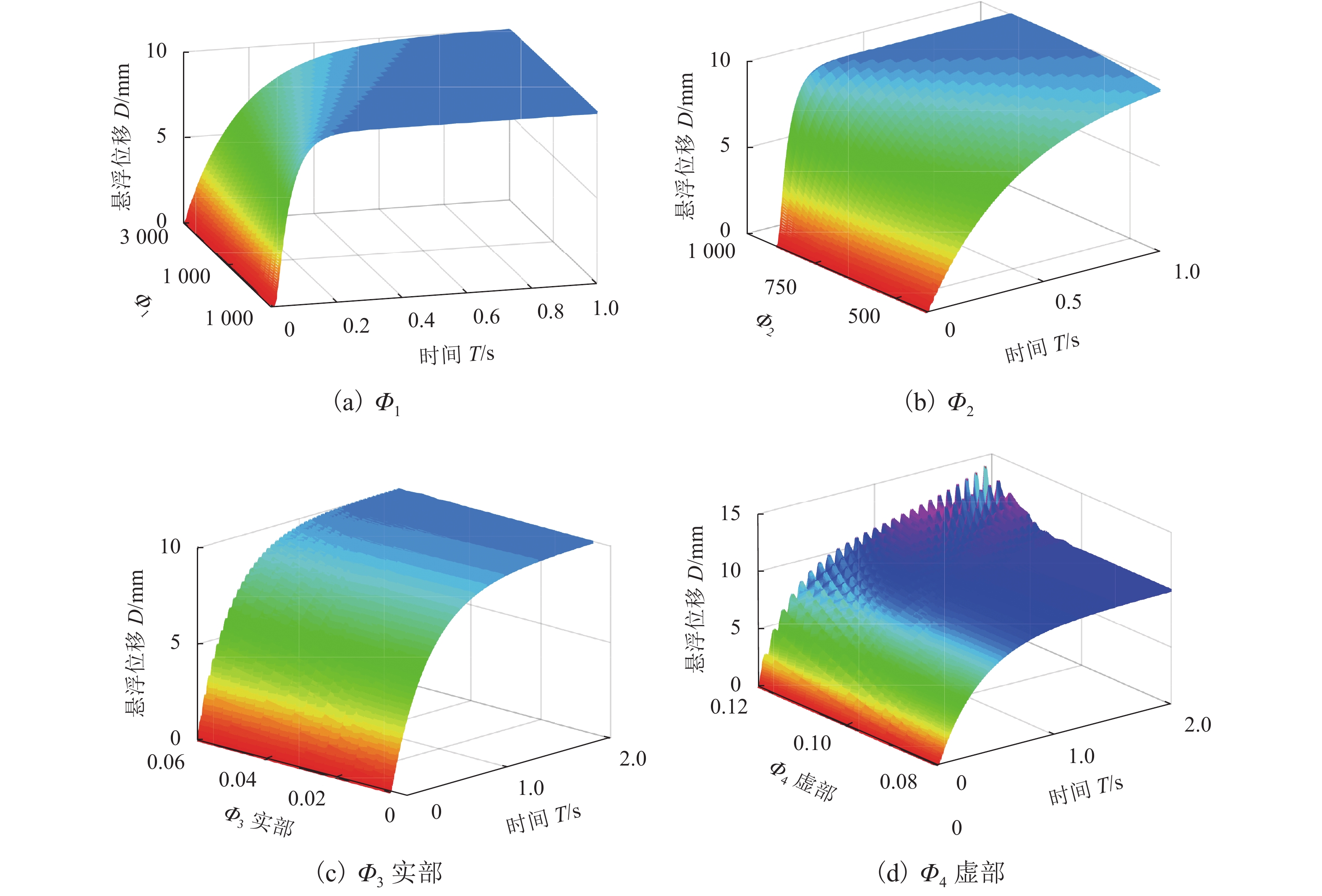
伪梯度Φ的初始值对悬浮效果的影响如图6所示,由图6可知:Φ1的减小或Φ2的增大会增大MFAC算法的响应速度,Φ3数值的变化不会影响系统的响应速度,但过大的Φ3会使系统发生震荡;伪梯度Φ的取值范围很大,有利于系统稳定性调节.
3.3 FFDL-MFAC与PID算法的控制效果对比
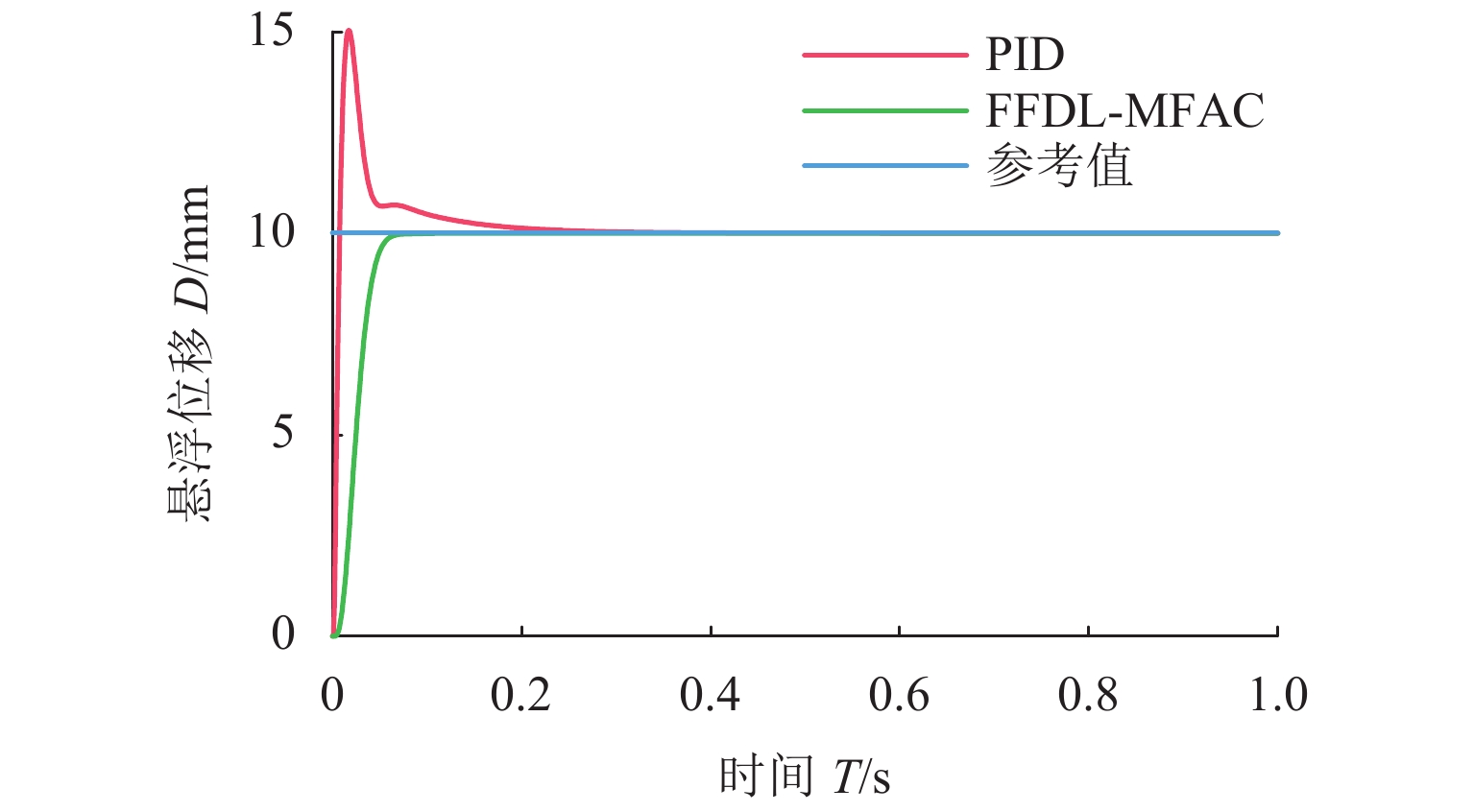
1) 阶跃信号的响应对比
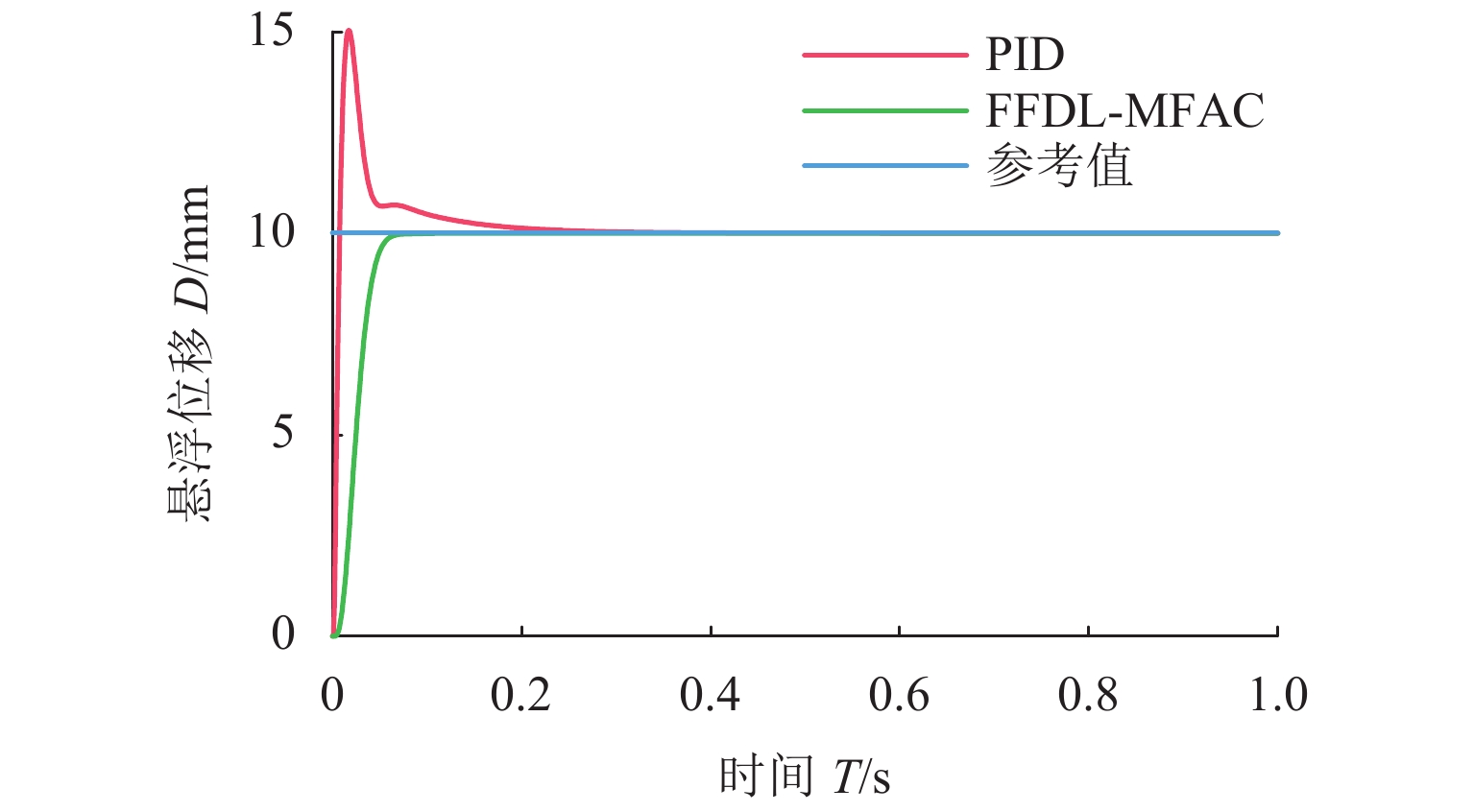
FFDL-MFAC与PID控制器对阶跃信号的响应如图7、表2所示. 可以看出:FFDL-MFAC对于阶跃信号的响应优于PID
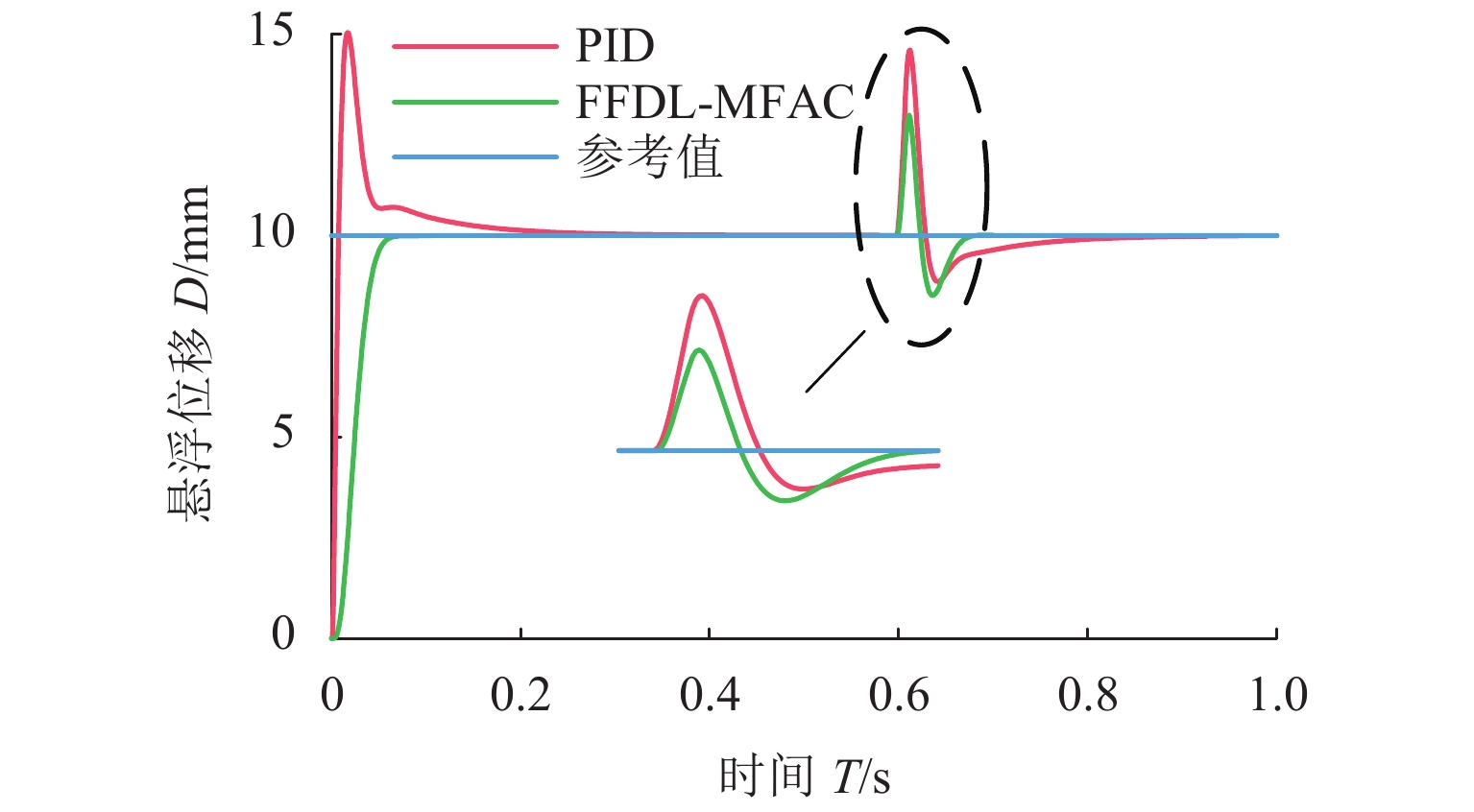
2) 干扰信号的响应对比
在单自由度磁悬浮系统中加入干扰信号d,
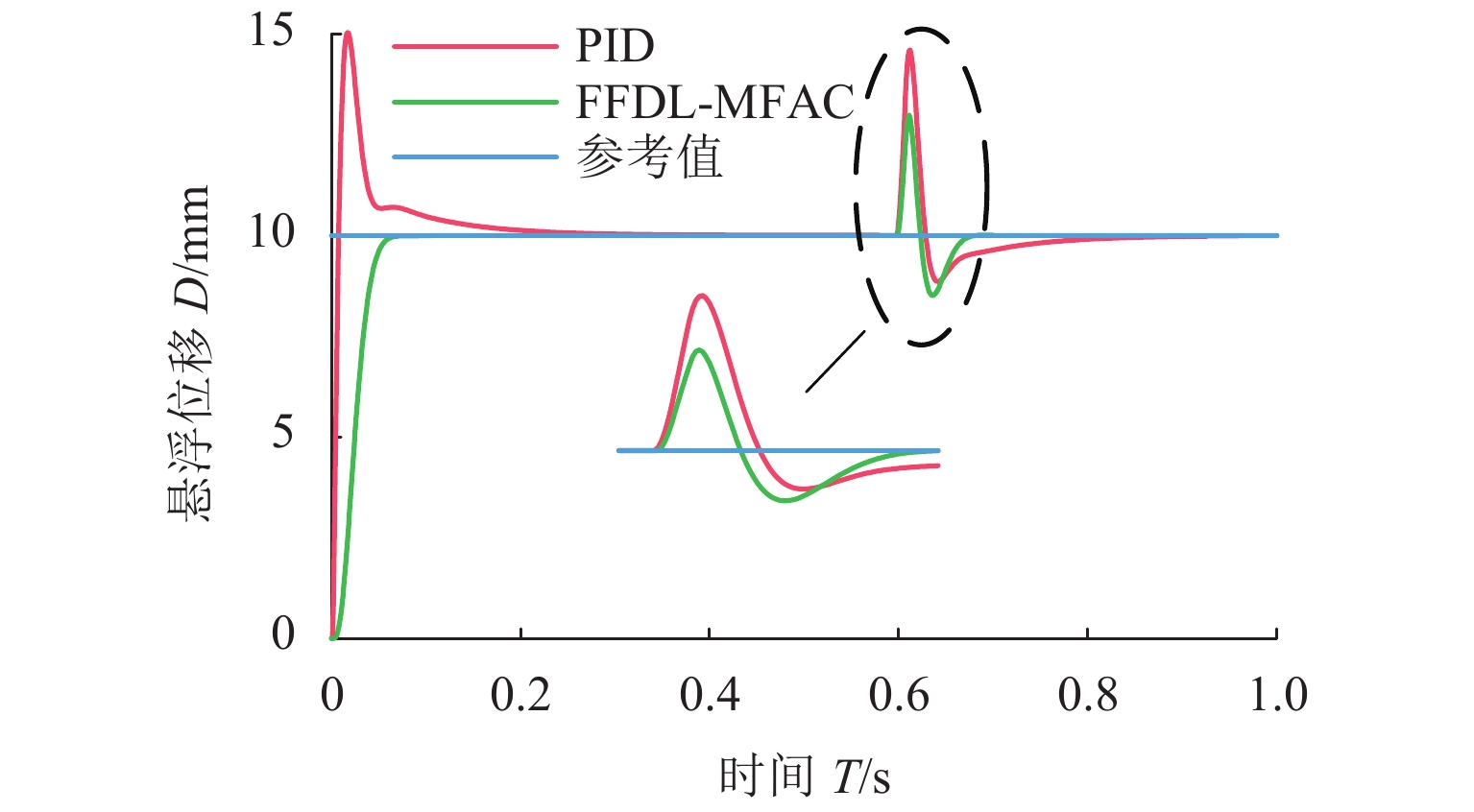
d(k) = \left\{ \begin{array}{l} 0,\quad k \leqslant 600, \\ 0.000\; 1,\quad 600 < k \leqslant 610, \\ 0,\quad k > 610 . \end{array} \right. (16) 则MFAC和PID控制器对干扰信号的响应如图8、表3所示. 对于干扰的抑制,MFAC的算法优于PID,超调量小,稳定快速.
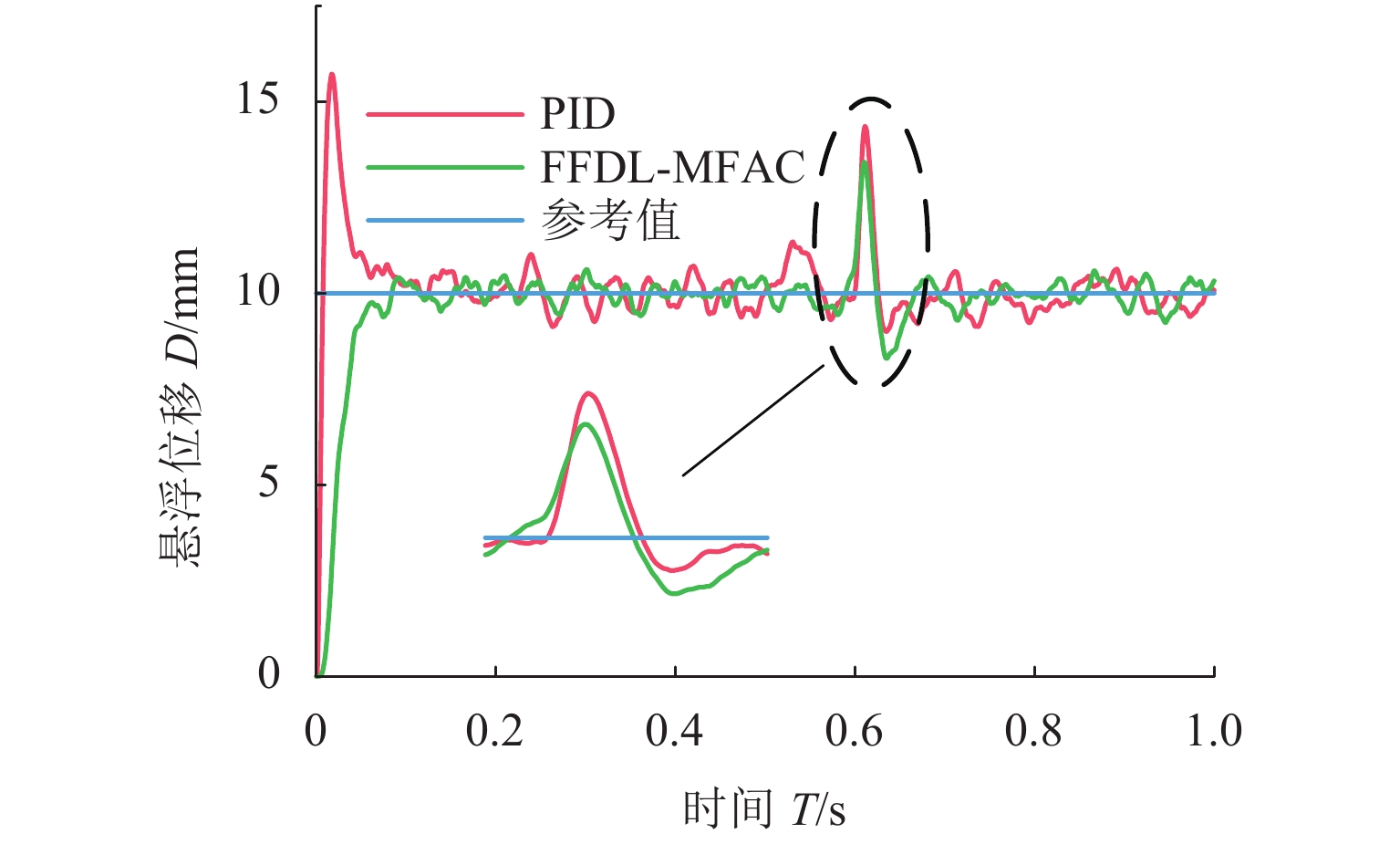
3) 噪声信号响应对比
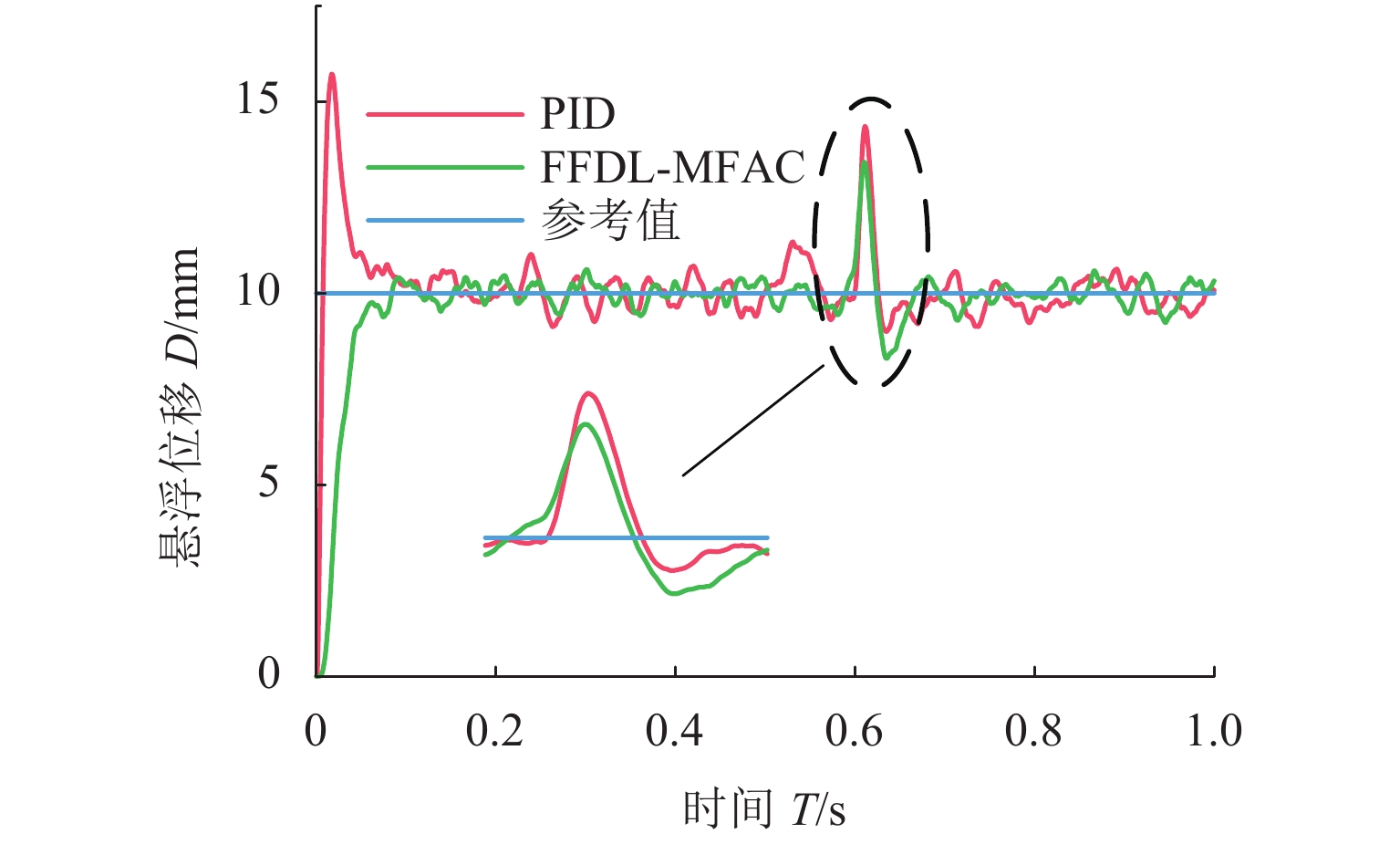
在单自由度磁悬浮系统中加入干扰信号d及噪声信号[−0.04, 0.04],则FFDL-MFAC和PID控制器仿真结果如图9. FFDL-MFAC与PID控制器的RMS分别为0.8422和1.388. 在含有噪声的情况下,FFDL-MFAC仍然对于干扰有很好的抑制作用.
表 2 阶跃信号响应下MFAC与PID控制器的性能对比Table 2. Performances comparison between MFAC and PID controller under step signal response控制器 超调量 稳定时间/s eRMS PID 0.005 0.205 1.2484 FFDL-MFAC 0 0.070 0.6350 表 3 干扰信号下MFAC与PID控制器的性能对比Table 3. Performance comparison between MFAC and PID controller under interfering signal response控制算法 eRMS PID 1.3341 FFDL-MFAC 0.7405 4. 实验分析
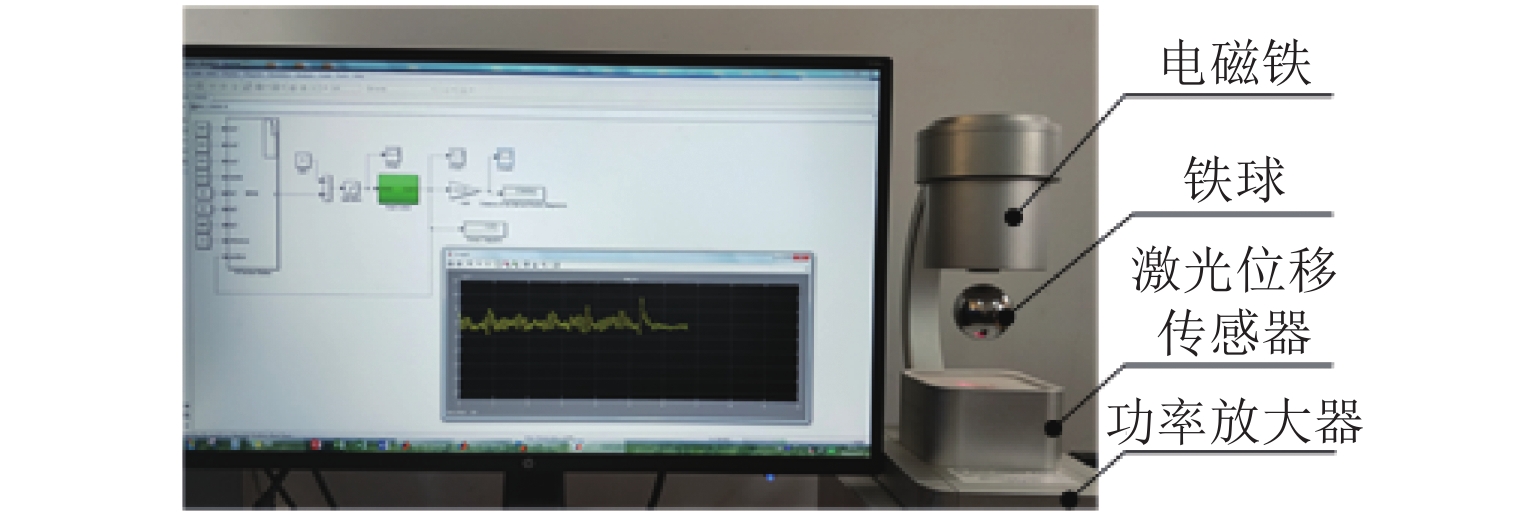
为验证FFDL-MFAC控制器对单自由度磁悬浮系统的控制效果,在单自由度磁悬浮实验平台上进行悬浮实验. 单自由度磁悬浮实验平台如图10所示,包含电磁铁、铁球、位移传感器、功率放大器及研华的控制板卡,其中由于板卡已经安装到计算机中,所以在图中并未标示出来. 自由度磁悬浮实验平台的参数见表1,其测量坐标如图11所示,以电磁铁吸附平面的中心为原点建立ZOX测量坐标系. 由几何关系可知,悬浮位移
{x_1} = {x_2} - {x_3} .悬浮实验中,同样采用FFDL-MFAC和PID算法进行对比悬浮实验,以误差均方根作为悬浮性能的评价指标. FFDL-MFAC与PID的实时控制程序分别如图12所示,通过PCI-1711输入模块与PCI-1711输出模块实现信号的输入与输出.
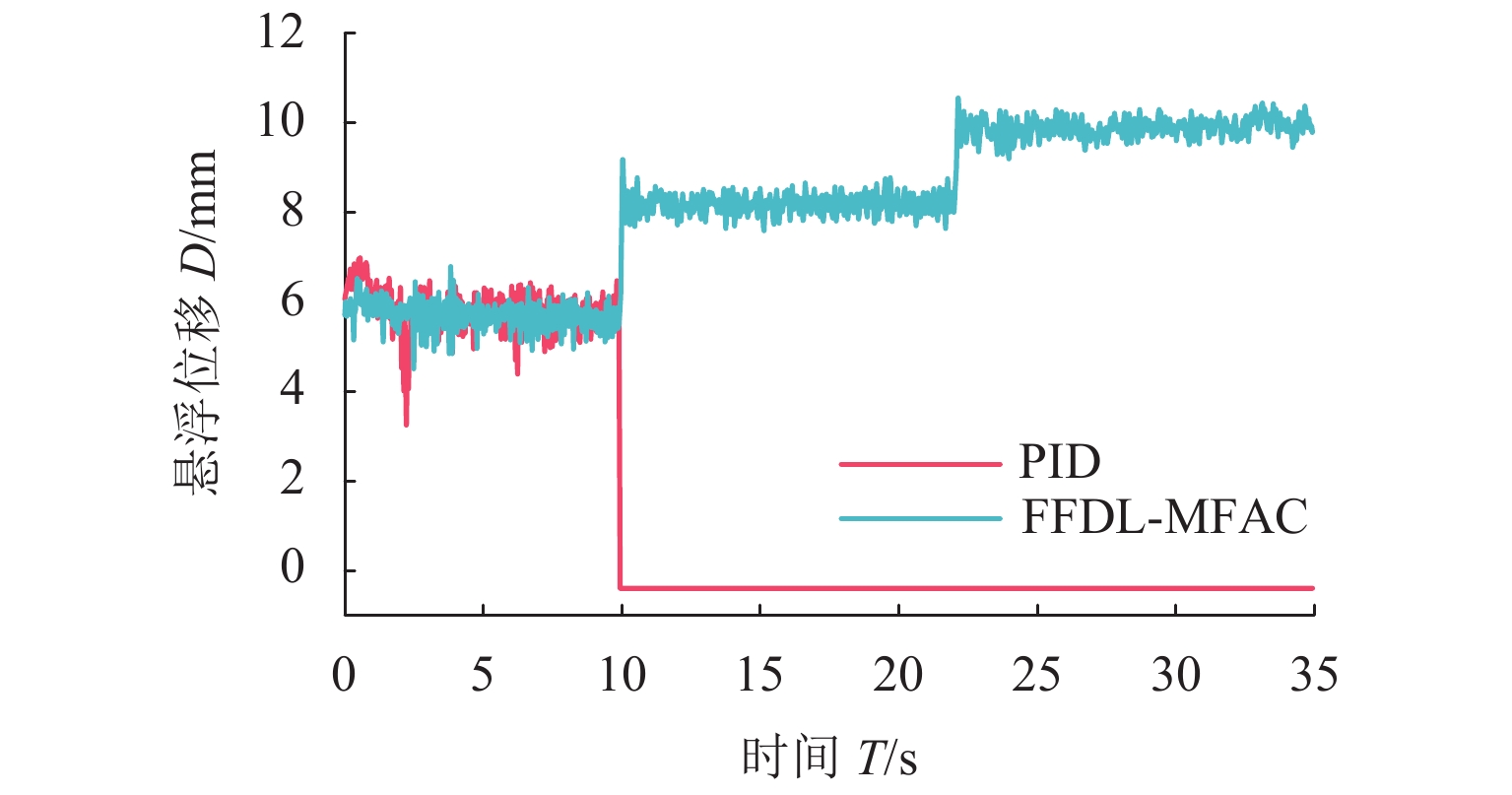
对于FFDL-MFAC控制器,其控制参数取Ly=1,Lu=2,μ=0.1,η=0.2,ρ=[0.6 0.6 1],λ=0.001, Φ(1)=[214000 21000 600]. PID控制器的最佳控制参数为Kp=100,Ki=0.0001,Kd=12. 两种算法的位置跟踪效果和悬浮效果如图13、14所示.
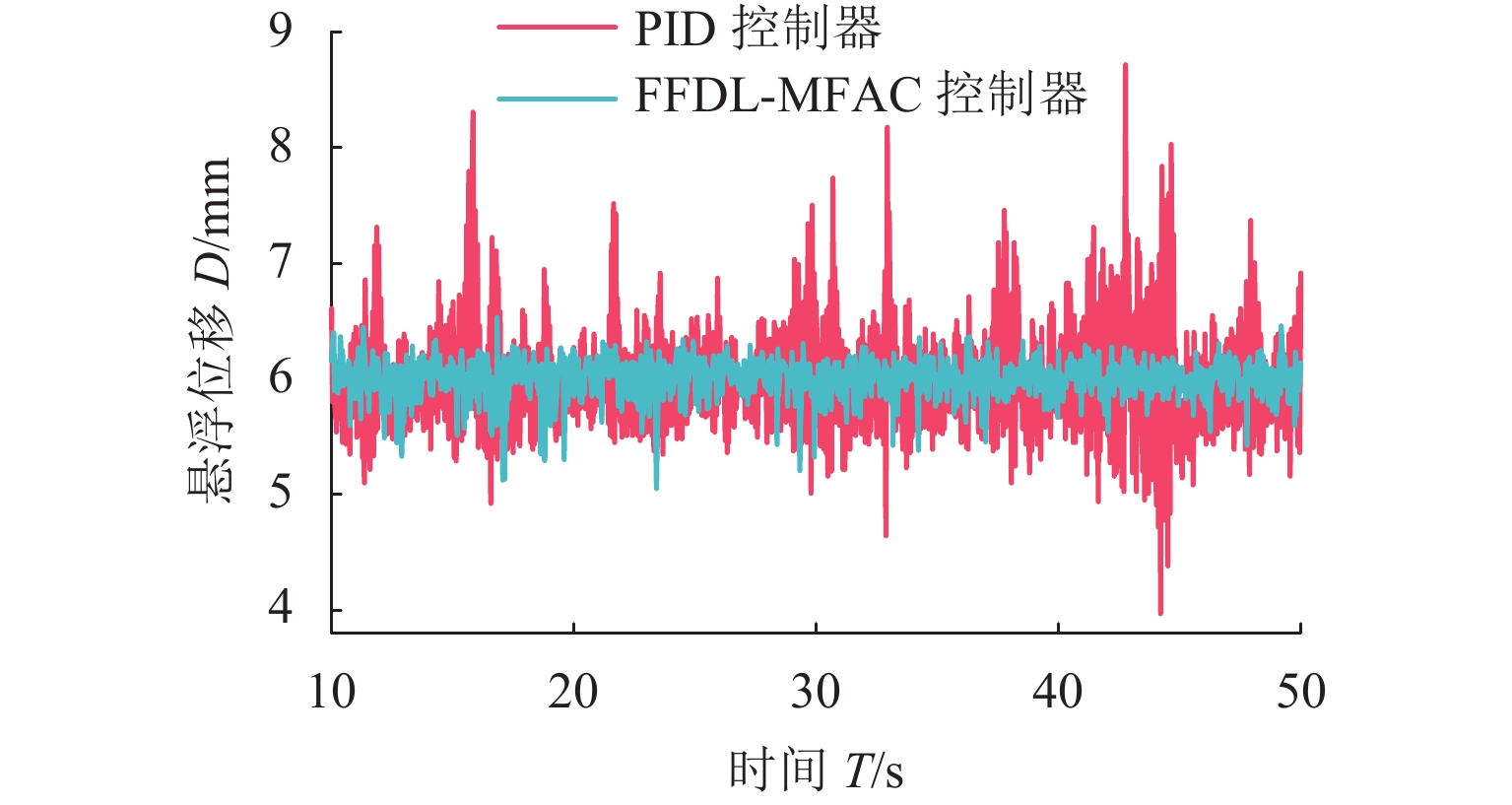
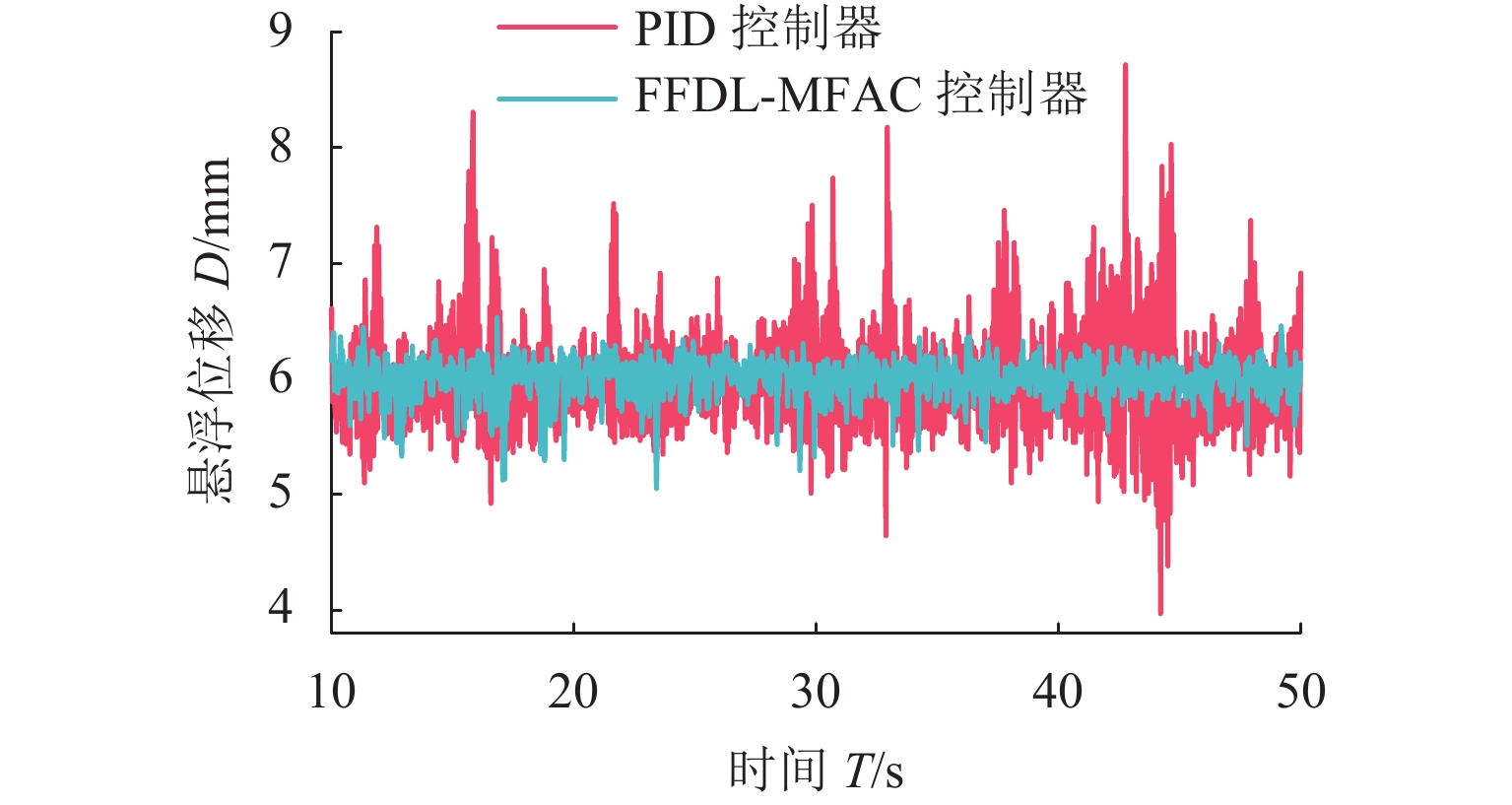
从图13、14可知:FFDL-MFAC算法具有很强的自适应性,在悬浮过程中,铁球的悬浮参考位置从6 mm变成8 mm和10 mm时,FFDL-MFAC能立即响应并快速实现铁球的稳定悬浮;而作为对比组的PID控制算法不具有自适应性,所以当悬浮参考位置发生变化时,铁球发生坠落.
从图14可知:FFDL-MFAC算法的悬浮精度优于PID,FFDL-MFAC算法的误差均方根为0.1542,而PID算法的误差均方根为0.4149.
5. 结 论
本文针对单自由度磁悬浮系统的非线性及精确数学模型难以建立的问题,通过引入FFDL-MFAC算法设计出适合单自由度磁悬浮系统的控制器. 仿真及实验结果表明,全格式无模型自适应控制方法只需采集单自由度磁悬浮系统在工作状态下的I/O数据,无需建立单自由度磁悬浮系统精确数学模型,通过设定全格式无模型自适应控制器参数即可实现高精度稳定悬浮控制,且控制器具有良好的自适应性和鲁棒性. 与传统PID相比,FFDL-MFAC具有以下优点:
1) FFDL-MFAC算法的控制精度高. 在悬浮过程中FFDL-MFAC算法的误差均方根为0.1542,而PID算法的误差均方根为0.4149.
2) FFDL-MFAC控制参数的调整域较大,有利于系统稳定性的调节.
-
表 1 单自由度磁悬浮系统的物理参数
Table 1. Physical parameters of a single-degree-of-freedom magnetically levitated system
符号 参数 值 m 小球质量/g 94 Ka 放大系数 6.508 A 系数 0.02012 B 系数/(A·m−1) 39.433 表 2 阶跃信号响应下MFAC与PID控制器的性能对比
Table 2. Performances comparison between MFAC and PID controller under step signal response
控制器 超调量 稳定时间/s eRMS PID 0.005 0.205 1.2484 FFDL-MFAC 0 0.070 0.6350 表 3 干扰信号下MFAC与PID控制器的性能对比
Table 3. Performance comparison between MFAC and PID controller under interfering signal response
控制算法 eRMS PID 1.3341 FFDL-MFAC 0.7405 -
[1] 禹春敏,邓智泉,梅磊,等. 基于精确磁路的新型混合型轴向-径向磁悬浮轴承研究[J]. 电工技术学报,2021,36(6): 1219-1228.YU Chunmin, DENG Zhiquan, MEI Lei, et al. Research of new hybrid axial-radial magnetic bearing based on accurate magnetic circuit[J]. Electrical Technology, 2021, 36(6): 1219-1228. [2] 李云钢,常文森. 磁浮列车悬浮系统的串级控制[J]. 自动化学报,1999,25(2): 247-251.LI Yungang, CHANG Wensen. Cascade control of an emsmaglev vehicle slevitatio control system[J]. Acta Automatica Sinica, 1999, 25(2): 247-251. [3] 王东,姜豪,苏振中,等. 船用磁悬浮轴承关键技术与发展综述[J]. 中国电机工程学报,2020,40(20): 6704-6715.WANG Dong, JIANG Hao, SU Zhenzhong, et al. A review on the key technologies and development of marine magnetic bearings[J]. Proceedings of the CSEE, 2020, 40(20): 6704-6715. [4] 张维煜,朱熀秋,袁野. 磁悬浮轴承应用发展及关键技术综述[J]. 电工技术学报,2015,30(12): 12-20.ZHANG Weiyu, ZHU Huangqiu, YUAN Ye. Study on keys and application of magnetic beartings[J]. Transaction of China Electrotechnical Society, 2015, 30(12): 12-20. [5] GERHARD S, MASLEN E H. Magnetic bearings-theory, design, and application to rotating machinery[M]. Berlin: Springer-Verlag, 2009: 1-17. [6] CHEN L L, ZHU C S, ZHONG Z X, et al. Internal model control for the AMB high-speed flywheel rotor system based on modal separation and inverse system method[J]. IET Electric Power Applications, 2019, 13(3): 349-358. doi: 10.1049/iet-epa.2018.5646 [7] CHEN L L, ZHU C S, ZHONG Z X, et al. Radial position control for magnetically suspended high-speed flywheel energy storage system with inverse system method and extended 2-DOF PID controller[J]. IET Electric Power Applications, 2020, 14(1): 71-81. doi: 10.1049/iet-epa.2019.0512 [8] CHEN S Y, SONG M H. Energy-saving dynamic bias current control of active magnetic bearing positioning system using adaptive differential evolution[J]. IEEE Transactions on Systems, Man, and Cybernetics: Systems, 2019, 49(5): 942-953. doi: 10.1109/TSMC.2017.2691304 [9] WEI C S, DIRK S. Optimization strategy for PID-controller design of AMB rotor systems[J]. IEEE Transactions on Control Systems Technology, 2016, 24(3): 788-803. doi: 10.1109/TCST.2015.2476780 [10] 吕治国,龙志强. 磁悬浮球系统的非线性自适应控制方法[J]. 控制工程,2020,27(1): 127-133.LYU Zhiguo, LONG Zhiqian. Method on nonlinear adaptive controller for maglev levitation ball system[J]. Control Engineering of China, 2020, 27(1): 127-133. [11] 朱坚民,沈正强,李孝茹. 基于神经网络反馈补偿控制的磁悬浮球位置控制[J]. 仪器仪表学报,2014,35(5): 976-986.ZHU Jianmin, SHEN Zhengqiang, LI Xiaoru. Magnetic levitation ball position control based on neural network feedback compensation control[J]. Chinese Journal of Scientific Instrument, 2014, 35(5): 976-986. [12] 张井岗,方线伟,赵志诚. 磁悬浮球系统的分数阶滑模控制[J]. 南京理工大学学报,2014,38(1): 72-77.ZHANG Jinggang, FANG Xianwei, ZHAO Zhicheng. Fractional order sliding mode control of magnetic levitation ball system[J]. Journal of Nanjing University of Science and Technology, 2014, 38(1): 72-77. [13] 宋荣荣,马卫华. 模糊PID控制在单自由度磁悬浮系统中的应用[J]. 重庆理工大学学报(自然科学),2014,28(5): 1-6.SONG Rongrong, MA Weihua. Application of fuzzy PID controller in the a single-degree-of-freedom magnetically levitated system[J]. Journal of Chongqing University of Technology (Natural Science), 2014, 28(5): 1-6. [14] 夏长峰,蔡远文,任元. 磁悬浮控制敏感陀螺转子偏转通道稳定控制方法[J]. 控制理论与应用,2020,37(7): 1535-1543.XIA Changfeng, CAI Yuanwen, REN Yuan. Stable control method for rotor tilt channel in magnetically suspended control and sensing gyro[J]. Control Theory & Applications, 2020, 37(7): 1535-1543. [15] HUO S W, ZHOU Y, LEI J J, et al. Iterative feedback control-based salient object segmentation[J]. IEEE Transactions on Multimedia, 2018, 20(6): 1350-1364. doi: 10.1109/TMM.2018.2831998 [16] ZHENG Y B, LIU X N, ZHAO J J, et al. A novel iterative learning control method and control system design for active magnetic bearing rotor imbalance of primary helium circulator in high-temperature gas-cooled reactor[J]. Measurement and Control, 2020, 53(3): 2029-2039. [17] CHILUKA S K, AMBATI S R, Seepana M M, et al. A novel robust virtual reference feedback tuning approach for minimum and non-minimum phase systems[J]. ISA Transactions, 2021, 115(5): 163-169. [18] 金尚泰,赵汝莉,侯忠生,等. 基于虚拟参考反馈整定的改进无模型自适应控制[J]. 控制与决策,2015,30(12): 66-67.JIN Shangtai, ZHAO Ruli, HOU Zhongsheng, et al. Improved model free adaptive control approach with virtual reference feedback tuning[J]. Control and Decision, 2015, 30(12): 66-67. [19] SCHAAL S, ATKESON C G. Robot juggling: implementation of memory-based learning[J]. IEEE Control Systems, 1994, 14(1): 57-71. doi: 10.1109/37.257895 [20] AHN H, CHEN Y Q, KEVIN L. Iterative learning control: brief survey and categorization[J]. IEEE Transactions on Systems, Man, and Cybernetics, Part C (Applications and Reviews), 2007, 37(6): 1099-1121. doi: 10.1109/TSMCC.2007.905759 [21] HOU Z S, JIN S T. A novel data-driven control approach for a class of discrete-time nonlinear systems[J]. IEEE Transactions on Control Systems Technology, 2011, 19(6): 1549-1558. doi: 10.1109/TCST.2010.2093136 [22] ZHANG X F, MA H B, ZHANG X H, et al. Compact model-free adaptive control algorithm for discrete-time nonlinear systems[J]. IEEE Access, 2019, 7(2): 141062-141071. [23] WANG Z, CHEN T W. Data and event-driven control of a class of networked non-linear control systems[J]. IET Control Theory and Applications, 2015, 9(7): 1034-1041. doi: 10.1049/iet-cta.2014.0711 [24] HOU Z S, JIN S T. Data-Driven model-free adaptive control for a class of MIMO nonlinear discrete-time systems[J]. IEEE Transactions on Neural Networks, 2011, 22(12): 2173-2183. doi: 10.1109/TNN.2011.2176141 [25] HOU Z S, XIONG S S. On model-free adaptive control and its stability analysis[J]. IEEE Transactions on Automatic Control, 2019, 64(11): 4555-4569. doi: 10.1109/TAC.2019.2894586 [26] JIANG Q Q, LIAO Y L, LI Y, et al. Heading control of unmanned surface vehicle with variable output constraint model-free adaptive control algorithm[J]. IEEE Access, 2019, 7(1): 131008-131018. [27] CHEN C, LU J G. Design of self-tuning SISO partial-form model-free adaptive controller for vapor-compression refrigeration system[J]. IEEE Access, 2019, 7(1): 125771-125782. [28] LU C, ZHAO Y, MEN K, et al. Wide-area power system stabiliser based on model-free adaptive control[J]. IET Control Theory & Applications, 2015, 9(13): 1996-2007. [29] LIU X, HOU Z S, JIN S T . Model-free adaptive control for magnetic levitation ball system[C]// Chinese Control Conference (CCC). [S.l.]: IEEE, 2012: 401-408. [30] 田涛涛,侯忠生,刘世达,等. 基于无模型自适应控制的无人驾驶汽车横向控制方法[J]. 自动化学报,2017(11): 66-75.TIAN Taotao, HOU Zhongsheng, LIU Shida, et al. Model-free adaptive control based lateral control of self-driving car[J]. Acta Automatica Sinica, 2017(11): 66-75. [31] 杨智,陈志堂,范正平,等. 基于改进粒子群优化算法的PID控制器整定[J]. 控制理论与应用,2010,27(10): 1346-1352.YANG Zhi, CHEN Zhitang, FAN Zhenping, et al. Tuning of PID controller based on improved particle-swarm-optimization[J]. Control Theory and Applications, 2010, 27(10): 1346-1352. 期刊类型引用(9)
1. 孙友刚,黄志创,林国斌,徐俊起,吉文. 高速磁浮车辆悬浮系统多元基神经网络容错控制. 交通运输工程学报. 2025(02): 61-74 .  百度学术
百度学术2. 徐通福,李秀英. 基于集中式卡尔曼滤波干扰观测器的无模型自适应控制. 机床与液压. 2024(01): 36-41 .  百度学术
百度学术3. 张振利,汪永壮,秦耀,杨杰. 基于Levant微分器的磁浮球控制算法研究. 系统仿真学报. 2024(07): 1586-1595 .  百度学术
百度学术4. 金俊杰,王岩峰,徐程程,陆文轩,张晓友,孙凤,徐方超. 人工肾脏泵用磁悬浮轴承设计与磁力特性分析. 西南交通大学学报. 2024(04): 795-803 .  本站查看
本站查看5. 刘怡,刘国清,酒芳恒,谌昊. 三轨电磁悬浮系统的模糊滑模控制研究. 电机与控制应用. 2024(10): 29-39 .  百度学术
百度学术6. 张静,陈伟,韩雪. 基于CarSim仿真的汽车底盘稳定性分析. 自动化与仪器仪表. 2023(03): 80-85+91 .  百度学术
百度学术7. 卢许孟,南新元,夏斯博. 无模型坐标补偿积分滑模约束的自动驾驶汽车轨迹跟踪控制. 广西师范大学学报(自然科学版). 2023(02): 36-48 .  百度学术
百度学术8. 杨杰,秦耀,汪永壮,张振利. 永磁电磁混合型磁浮球的改进滑模控制方法. 湖南大学学报(自然科学版). 2023(04): 200-209 .  百度学术
百度学术9. 金超武,曹迎庆,周瑾,叶周铖,辛宇. 采用模型辅助ESO的磁悬浮转子抗干扰性能. 西南交通大学学报. 2023(04): 870-878 .  本站查看
本站查看其他类型引用(10)
-






 下载:
下载:
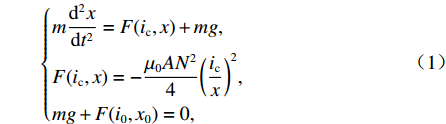
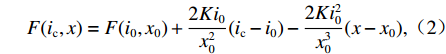
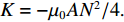
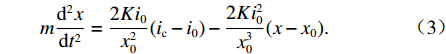


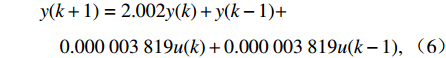

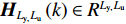
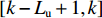
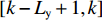
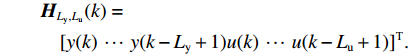
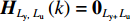
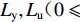

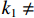
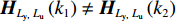
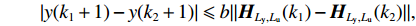
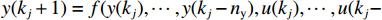
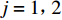
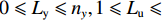
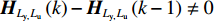
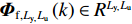
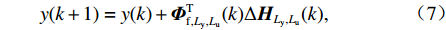
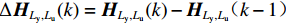

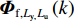

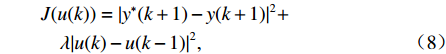

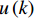
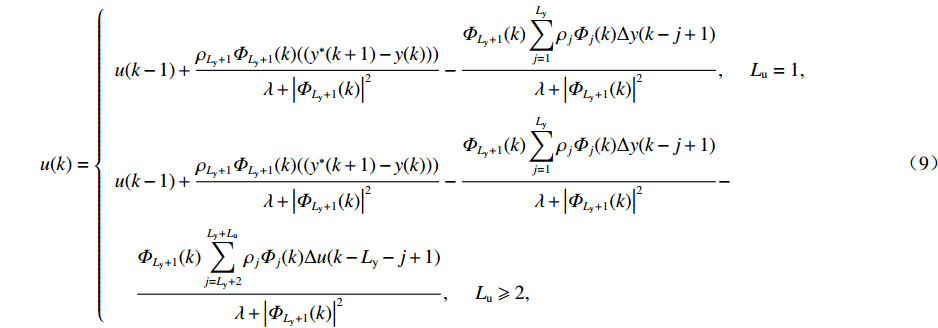


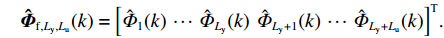
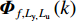
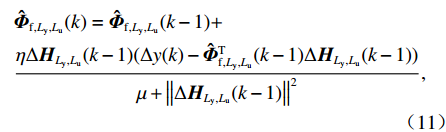
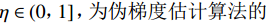
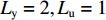
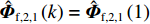
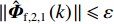
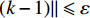
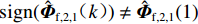
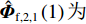
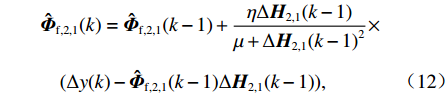
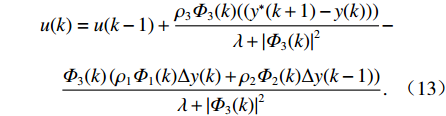
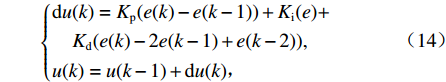

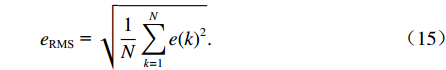
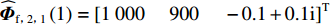


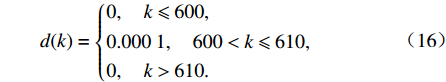












 下载:
下载:




 百度学术
百度学术