Analysis of Longitudinal Deformation of Shield Tunnel Structures with Consideration of Axial Force and Shear Effect
-
摘要:
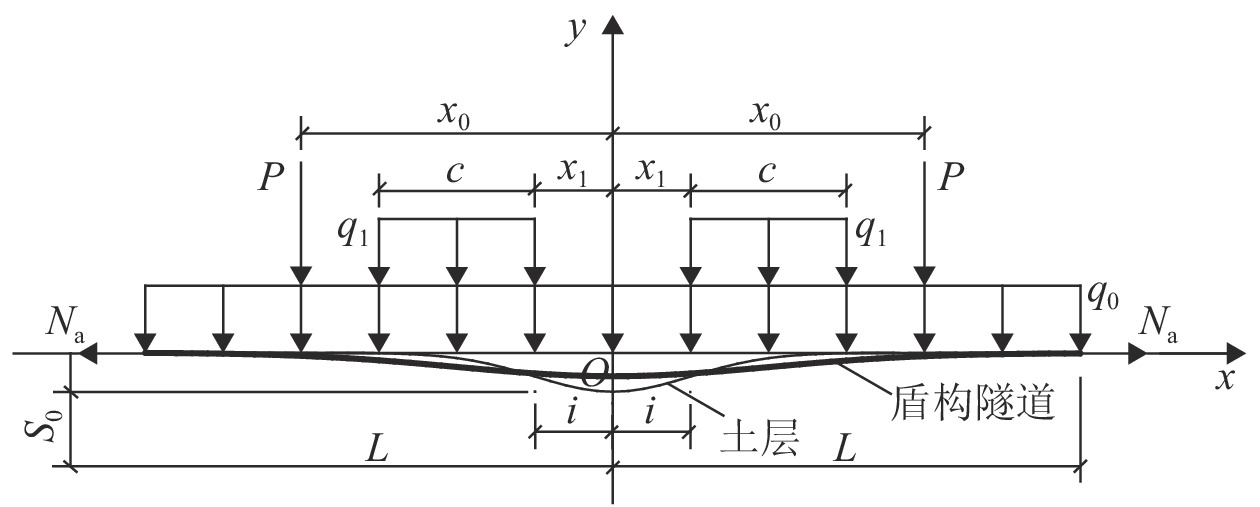
采用傅立叶级数法研究了不同荷载作用下轴力和剪切效应对盾构隧道变形的影响. 计及剪切变形所产生的地基反力，建立了弯曲变形的控制微分方程，推导了剪切变形的计算公式；采用与既有理论解对比的方法，验证了级数解的正确性；通过对比计算，分析了截面形式、端承条件、荷载形式、长高比以及有无弹性地基对盾构隧道剪切变形的影响，剪切刚度对弯曲变形、剪切变形及内力的影响，以及装配力对弯曲变形和弯曲内力的影响. 研究结果表明：盾构隧道的剪切变形在总变形中的占比可以达到20%以上，是由于盾构隧道圆环截面的形式及较低的剪切刚度共同造成的；考虑剪切变形后，隧道的总变形是增加的，但弯曲变形及弯矩较不考虑剪切变形时要小；随剪切刚度的降低，剪切变形增大，其在总变形中的占比也增大，当剪切刚度从8 GN减小到1 GN，总变形增加了15.7%，弯曲变形及弯矩分别减小了11.7%、17.1%，剪切变形增加5.77倍，剪切变形在总变形中的占比从4.64%增加到27.17%；装配产生的轴向压力增大了隧道的弯曲变形和弯矩，但影响幅度不大，一般对挠度的影响不超过2%，对弯矩的影响不超过3%.
Abstract:The influence of axial force and shear effect on the longitudinal deformation of shield tunnel structures under different loads is investigated by Fourier series method. Taking into account the foundation reaction caused by shear deformation, the governing differential equation of bending deformation is derived, and a formula is proposed to calculate the shear deformation. The correctness of the Fourier series solution is verified by comparison with analytical solutions. Through comparative calculation, the main work is focused on addressing the influence of cross section, end support, type of load, ratio of length to height, and elastic foundation on the shear deformation of shield tunnel, the influence of shear rigidity on bending deformation, shear deformation and internal forces, and the influence of axial load exerted by installation of segment rings on bending deformation and bending moment. Results show that the shear deformation of shield tunnel accounts for more than 20% of the total deformation, which is caused by the form of annular section and low shear rigidity of shield tunnel. When shear deformation is counted, the whole deformation gets larger, but the bending deformation and bending moment are smaller than those without consideration of shear deformation. As shear rigidity decreases, shear deformation increases, and its proportion to the entire deformation also increases. In our case studies, when the shear rigidity decreases from 8×106 to 1×106 kN, the whole deformation goes up by 15.7%; the bending deformation and bending moment go down by 11.7% and 17.1%, respectively; the shear deformation increases 5.77 times; and the percentage of shear deformation to the whole deformation increases from 4.64% to 27.17%. Besides, the bending deformation and bending moment are also increased by installation-caused axial load, but the influence range is limited, often less than 2% in deflection and less than 3% in bending moment.
-
Key words:
- shield tunnel /
- longitudinal deformation /
- shear effect /
- axial load and force /
- Fourier series /
- elastic foundation
-
图 1 盾构隧道上的作用荷载及其沉降示意
Figure 1. Schematic of loads on a shield tunnel and its deflection
表 1 无弹性地基时解析解与傅立叶级数解的计算结果
Table 1. Analytical and Fourier series solutions for an ordinary beam
序号 梁类型 荷载 Euler-Bernoulli梁 Timoshenko 梁 计算式 解析解计算值 级数解计算值 计算式[10] 解析解计算值 级数解计算值 例 1 两端简
支梁满布均
布荷载${M_{\rm{c} } } = \dfrac{ { {q_0}{l^2} } }{ {\rm{8} } }$ 24 025.00 24 025.00 ${M_{\rm{c} } } = \dfrac{ { {q_0}{l^2} } }{ {\rm{8} } }$ 24 025.00 24 025.00 ${w_{\rm{c} } } = \dfrac{ {5{q_0}{l^4} } }{ {384EI} }$ 120.250 00 120.250 00 ${w_{\rm{c}}} = \dfrac{{5{q_0}{l^4}}}{{384EI}} + \dfrac{{{M_{\rm{c}}}}}{{\eta GA}}$ 126.256 00 126.256 00 例 2 两端简
支梁跨中集
中荷载${M_{\rm{c} } } = \dfrac{ {Pl} }{ {\rm{4} } }$ 15 500.00 15 484.30 ${M_{\rm{c} } } = \dfrac{ {Pl} }{ {\rm{4} } }$ 15 500.00 15 484.30 ${w_{\rm{c}}} = \dfrac{{P{l^3}}}{{48EI}}$ 62.064 60 62.064 30 ${w_{\rm{c}}} = \dfrac{{P{l^3}}}{{48EI}} + \dfrac{{{M_{\rm{c}}}}}{{\eta GA}}$ 65.939 60 65.935 40 例 3 两端固
支梁满布均
布荷载${M_{\rm{c} } } = \dfrac{ { {q_0}{l^2} } }{ { {\rm{24} } } }$ 8 008.33 8 008.33 ${M_{\rm{c} } } = \dfrac{ { {q_0}{l^2} } }{ { {\rm{24} } } }$ 8 008.33 8 008.33 ${w_{\rm{c} } } = \dfrac{ {{q_0}{l^4} } }{ {384EI} }$ 24.050 00 24.050 00 ${w_{\rm{c}}} = \dfrac{{{q_0}{l^4}}}{{384EI}} + \dfrac{{{\rm{3}}{M_{\rm{c}}}}}{{\eta GA}}$ 30.056 30 30.056 30 例 4 两端固
支梁跨中集
中荷载${M_{\rm{c} } } = \dfrac{ {Pl} }{ {\rm{8} } }$ 7 750.00 7 734.34 ${M_{\rm{c} } } = \dfrac{ {Pl} }{ {\rm{8} } }$ 7 750.00 7 734.34 ${w_{\rm{c} } } = \dfrac{ {P{l^3} } }{ {192EI} }$ 15.516 10 15.516 10 ${w_{\rm{c}}} = \dfrac{{P{l^3}}}{{192EI}} + \dfrac{{2{M_{\rm{c}}}}}{{\eta GA}}$ 19.391 10 19.387 20 
下载: 导出CSV
表 2 有弹性地基时解析解与傅立叶级数解的计算结果
Table 2. Analytical and Fourier series solutions for a beam on elastic foundation
序号 梁类型 荷载 计算式[5] 解析解
计算值级数解
计算值例 5 无限长梁 跨中局部
均布荷载${M_{\rm{c} } } = \dfrac{ {q_{1} } }{ {2{\lambda ^2} } }{ {\rm{e} }^{ - \lambda c} }\sin \lambda c $ 2 655.02 2 655.06 ${w_{\rm{c} } } = \dfrac{ {q_{1} } }{ {KD} }(1 - { {\rm{e} }^{ - \lambda c} }\cos \lambda c)$ 5.184 58 5.184 06 例 6 有限长梁，
两端自由跨中集
中荷载${M_{\rm{c} } } = \dfrac{P}{ { {\rm{4} }\lambda } }\dfrac{ {\cosh \lambda L - \cos \lambda L} }{ {\sinh \lambda L + \sin \lambda L} }$ 3 946.29 3 930.62 ${w_{\rm{c} } } = \dfrac{ {P\lambda } }{ { { {2KD} } } }\dfrac{ {\cosh \lambda L + \cos \lambda L + 2} }{ {\sinh \lambda L + \sin \lambda L} }$ 5.760 30 5.760 28 ${w_{\rm{d}}} = \dfrac{{{\rm{2}}P\lambda }}{{{{KD}}}}\dfrac{{\cosh \dfrac{{\lambda L}}{{\rm{2}}}\cos \dfrac{{\lambda L}}{{\rm{2}}}}}{{\sinh \lambda L + \sin \lambda L}}$ −1.335 42
（向上）−1.335 36
（向上）例 7 无限长梁 跨中集中
荷载及
轴向压力${M_{\rm{c} } } = \dfrac{P}{ {\rm{4} } } \times \dfrac{1}{\alpha }$ 3 795.20 3 756.09 ${w_{\rm{c} } } = \dfrac{P}{ {2KD} } \times \dfrac{ { {\lambda ^2} } }{\alpha }$ 5.388 83 5.388 27 例 8 有限长梁，
两端简支满布均布
荷载及
轴向压力${M_{\rm{c} } } = \dfrac{ {EI{q_0} } }{ { { {KD} } } }\dfrac{1}{ {2\alpha \beta (\cosh \alpha L + \cos \beta L)} }\; \times$
$\left\{ {2\alpha \beta \left[ {2({\alpha ^2} - {\beta ^2})\cosh \dfrac{{\alpha L}}{2}\cos \dfrac{{\beta L}}{2} + 4\alpha \beta \sinh \dfrac{{\alpha L}}{2}\sin \dfrac{{\beta L}}{2}} \right] + } \right.$
$\left. {({\alpha ^2} - {\beta ^2})\left[ {2({\alpha ^2} - {\beta ^2})\sinh \dfrac{ {\alpha L} }{2}\sin \dfrac{ {\beta L} }{2} - 4\alpha \beta \cosh \dfrac{ {\alpha L} }{2}\cos \dfrac{ {\beta L} }{2} } \right]} \right\}$1 294.74 1 294.74 ${w_{\rm{c}}} = \dfrac{{q_0}}{{KD}}\left\{ {1 - \dfrac{1}{{2\alpha \beta (\cosh \alpha L + \cos \beta L)}} \times } \right.$
$\left. {\left[ {4\alpha \beta \cosh \dfrac{{\alpha L}}{2}\cos \dfrac{{\beta L}}{2} + {\rm{2}}({\alpha ^2} - {\beta ^2})\sinh \dfrac{{\alpha L}}{2}\sin \dfrac{{\beta L}}{2}} \right]} \right\}$9.130 38 9.130 38 注：$\lambda = \sqrt[\uproot{10}{\scriptstyle{4}}]{{\dfrac{{KD}}{{4EI}}}}$；$\alpha = \sqrt {{\lambda ^2} + \dfrac{N}{{4EI}}} $；$\beta = \sqrt {{\lambda ^2} - \dfrac{N}{{4EI}}} $.
下载: 导出CSV
表 3 截面形式对比计算结果
Table 3. Comparison between circular and annular cross sections
算例 端承条件 荷载形式 截面形式 中点弯曲挠度/mm 中点剪切挠度/mm 总挠度/mm 剪切挠度与总挠度之比/% 1 两端简支 满布均布荷载 圆截面 3.844 320 0.063 050 3.907 370 1.61 2 两端简支 满布均布荷载 圆环截面 10.097 400 0.495 100 10.592 500 4.67 3 两端固支 集中荷载 圆截面 0.496 042 0.040 675 0.536 717 7.58 4 两端固支 集中荷载 圆环截面 1.302 890 0.319 440 1.622 330 19.69
下载: 导出CSV
表 4 盾构隧道弯曲变形和剪切变形的计算结果
Table 4. Bending and shear deformations of shield tunnels
端承条件 荷载形式 l/D 中点弯曲
变形/mm中点剪切
变形/mm总变形/mm 剪切变形与
总变形之比/%两端自由 土层位移荷载 10 4.550 13 0.460 413 5.010 54 9.19 集中荷载 5.431 39 0.945 282 6.376 67 14.82 两端简支 满布均布荷载 10 8.660 22 0.309 989 8.970 21 3.46 土层位移荷载 4.489 47 0.428 843 4.918 31 8.72 集中荷载 5.338 90 0.897 159 6.236 06 14.39 两端固支 满布均布荷载 10 5.766 36 1.654 450 7.420 81 22.29 土层位移荷载 3.652 47 0.817 705 4.470 18 18.29 集中荷载 4.499 62 1.287 080 5.786 70 22.24 无限长梁 土层位移荷载 10 4.303 52 0.416 186 4.719 71 8.82 集中荷载 5.139 85 0.882 564 6.022 41 14.65 两端自由 集中荷载 20 5.131 45 0.894 990 6.026 44 14.85 30 5.129 50 0.891 378 6.020 88 14.80 两端简支 集中荷载 20 5.127 17 0.895 665 6.022 84 14.87 30 5.129 44 0.891 370 6.02 0 81 14.80 两端固支 集中荷载 15 5.090 32 0.921 379 6.011 70 15.33 20 5.156 69 0.862 406 6.019 10 14.33 30 5.131 48 0.889 306 6.020 79 14.77
下载: 导出CSV
表 5 无弹性地基时弯曲变形和剪切变形的计算结果
Table 5. Bending and shear deformations of a shield tunnel as an ordinary beam without elastic foundation
端承条件 荷载形式 中点弯曲变形/mm 中点剪切变形/mm 总变形/mm 剪切变形与总变形之比/% 两端简支 满布均布荷载 120.250 00 6.006 25 126.256 00 4.76 两端简支 集中荷载 62.064 30 3.871 07 65.935 40 5.87 两端固支 满布均布荷载 24.050 00 6.006 25 30.056 30 19.98 两端固支 集中荷载 15.516 10 3.871 07 19.387 20 19.97
下载: 导出CSV
表 6 盾构隧道剪切刚度对变形影响的计算结果
Table 6. Influence of shear rigidity of a shield tunnel on its deformation
剪切刚度/MN 中点弯曲变形/mm 中点剪切变形/mm 总变形/mm 剪切变形与总变形之比/% 中点弯矩/(kN•m) ∞ 4.472 68 0 4.472 68 0 1 807.01 8 000 4.386 15 0.213 643 4.599 79 4.64 1 752.17 4 000 4.303 52 0.416 186 4.719 71 8.82 1 700.96 2 000 4.148 76 0.791 742 4.940 50 16.03 1 607.98 1 000 3.874 60 1.445 400 5.320 00 27.17 1 452.24
下载: 导出CSV
表 7 盾构隧道装配轴力对变形影响的计算结果
Table 7. Influence of installation caused axial force of a shield tunnel on its deformation
轴向压力/MN 中点变形/mm 挠度较无轴力时增加的幅度/% 中点弯矩/（kN•m） 弯矩较无轴力时增加的幅度/% 0 6.505 34 0 1 909.40 0 10 6.525 95 0.32 1 919.77 0.54 20 6.546 78 0.64 1 930.27 1.09 40 6.589 10 1.29 1 951.64 2.21
下载: 导出CSV
-
[1] 廖少明,门燕青,肖明清,等. 软土盾构法隧道纵向应力松弛规律的实测分析[J]. 岩土工程学报,2017,39(5): 795-803. doi: 10.11779/CJGE201705003LIAO Shaoming, MEN Yanqing, XIAO Mingqing, et al. Field tests on longitudinal stress relaxation along shield tunnel in soft ground[J]. Chinese Journal of Geotechnical Engineering, 2017, 39(5): 795-803. doi: 10.11779/CJGE201705003 [2] TIMOSHENKO S P. Strength of materials, part I, elementary theory and problems[M]. Third edition. New York: D. Van Nostrand Company Inc., 1955. [3] WU H N, SHEN S L, LIAO S M, et al. Longitudinal structural modelling of shield tunnels considering shearing dislocation between segmental rings[J]. Tunnelling and Underground Space Technology, 2015, 50(8): 317-323. [4] 梁荣柱,林存刚,夏唐代,等. 考虑隧道剪切效应的基坑开挖对邻近隧道纵向变形分析[J]. 岩石力学与工程学报,2017,36(1): 223-233.LIANG Rongzhu, LIN Cungang, XIA Tangdai, et al. Analysis on the longitudinal deformation of tunnels due to pit excavation considering the tunnel shearing effect[J]. Chinese Journal of Rock Mechanics and Engineering, 2017, 36(1): 223-233. [5] HETENYI M. Beams on elastic foundation[M]. Ann Arbor: University of Michigan Press, 1946. [6] 杨成永,程霖,余乐,等. 隧道沉降引起的轨道结构变形与脱空[J]. 中国铁道科学,2018,39(6): 37-43. doi: 10.3969/j.issn.1001-4632.2018.06.06YANG Chengyong, CHENG Lin, YU Le, et al. Deformation and cavity of track structure due to tunnel settlement[J]. China Railway Science, 2018, 39(6): 37-43. doi: 10.3969/j.issn.1001-4632.2018.06.06 [7] COWPER G R. The shear coefficient in Timoshenko’s beam theory[J]. Journal of Applied Mechanics, 1966, 33(2): 335-340. doi: 10.1115/1.3625046 [8] HUTCHINSON J R. Shear coefficients for Timoshenko beam theory[J]. Journal of Applied Mechanics, 2001, 68(1): 87-92. doi: 10.1115/1.1349417 [9] 严宗达. 结构力学中的富里叶级数解法[M]. 天津: 天津大学出版社, 1989. [10] WANG C M. Timoshenko beam-bending solutions in terms of Euler-Bernoulli solutions[J]. Journal of Engineering Mechanics, 1995, 121(6): 763-765. doi: 10.1061/(ASCE)0733-9399(1995)121:6(763) -
下载:
点击查看大图
计量
- 文章访问数: 477
- HTML全文浏览量: 226
- PDF下载量: 33
- 被引次数: 0