Seismic Response of Continuous Beam-Arch Bridge under Spatially Varying Ground Motions
-
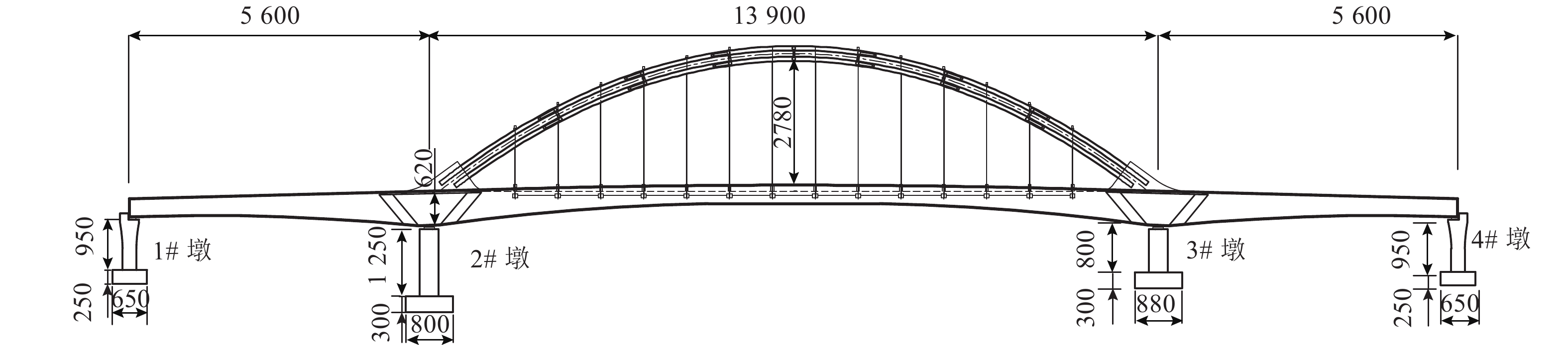
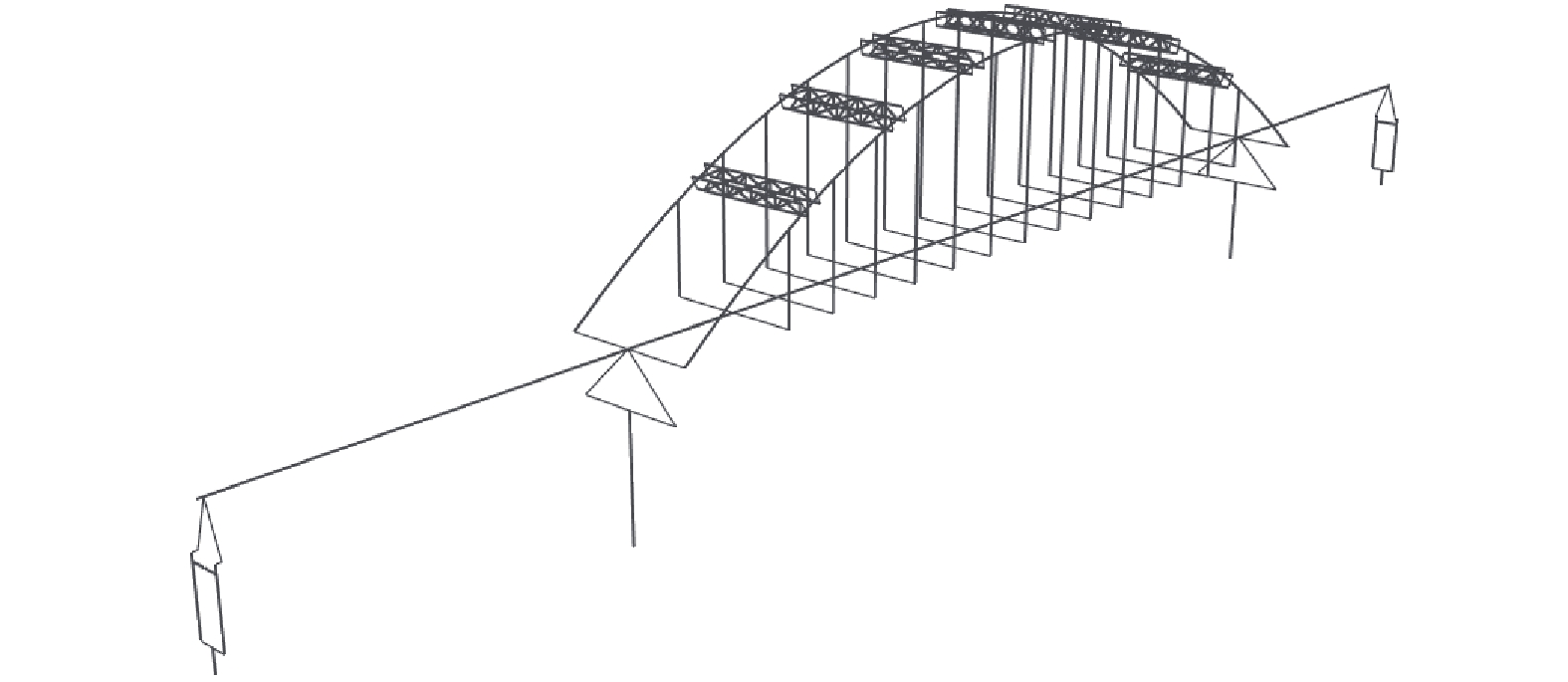
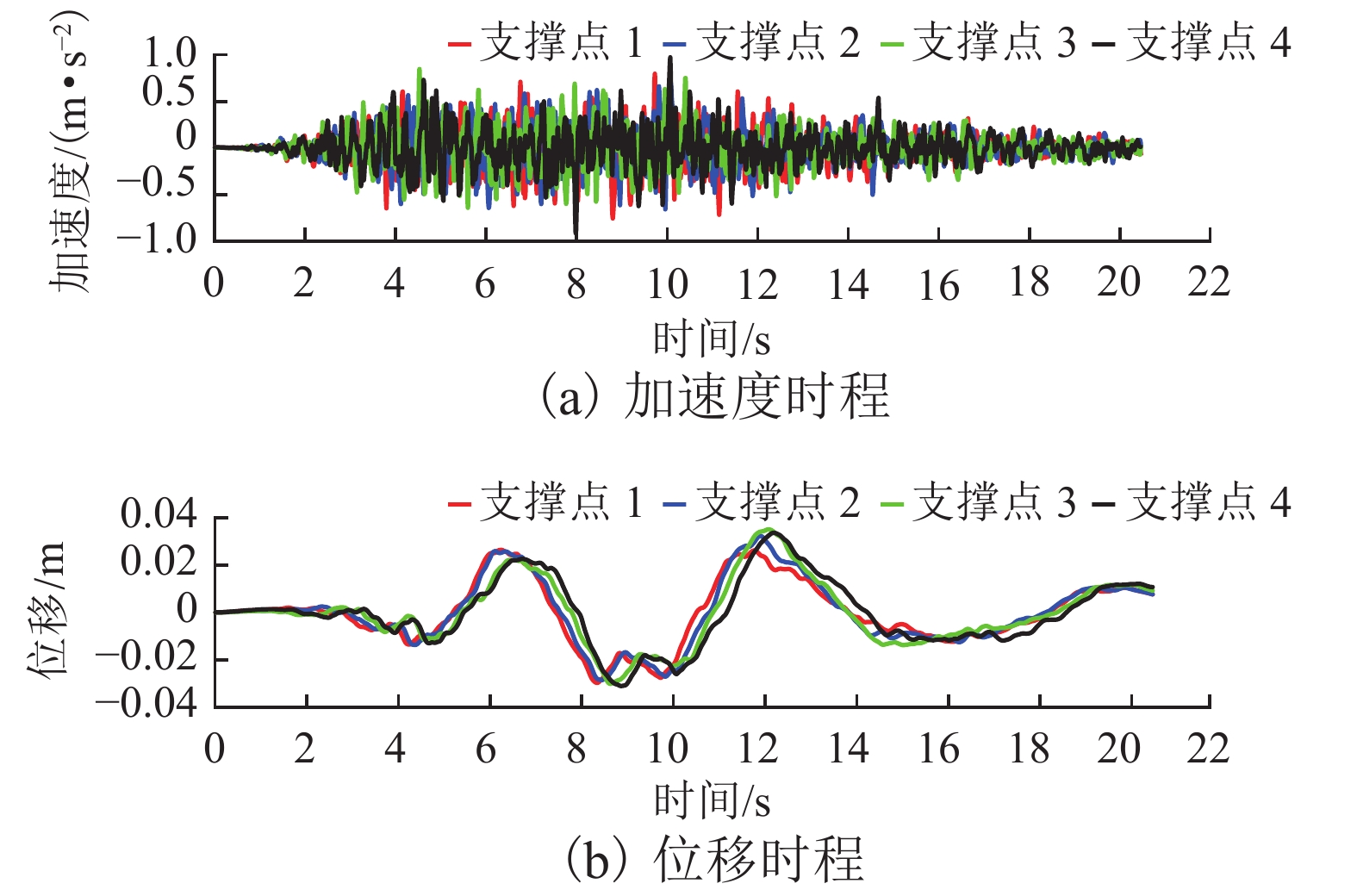
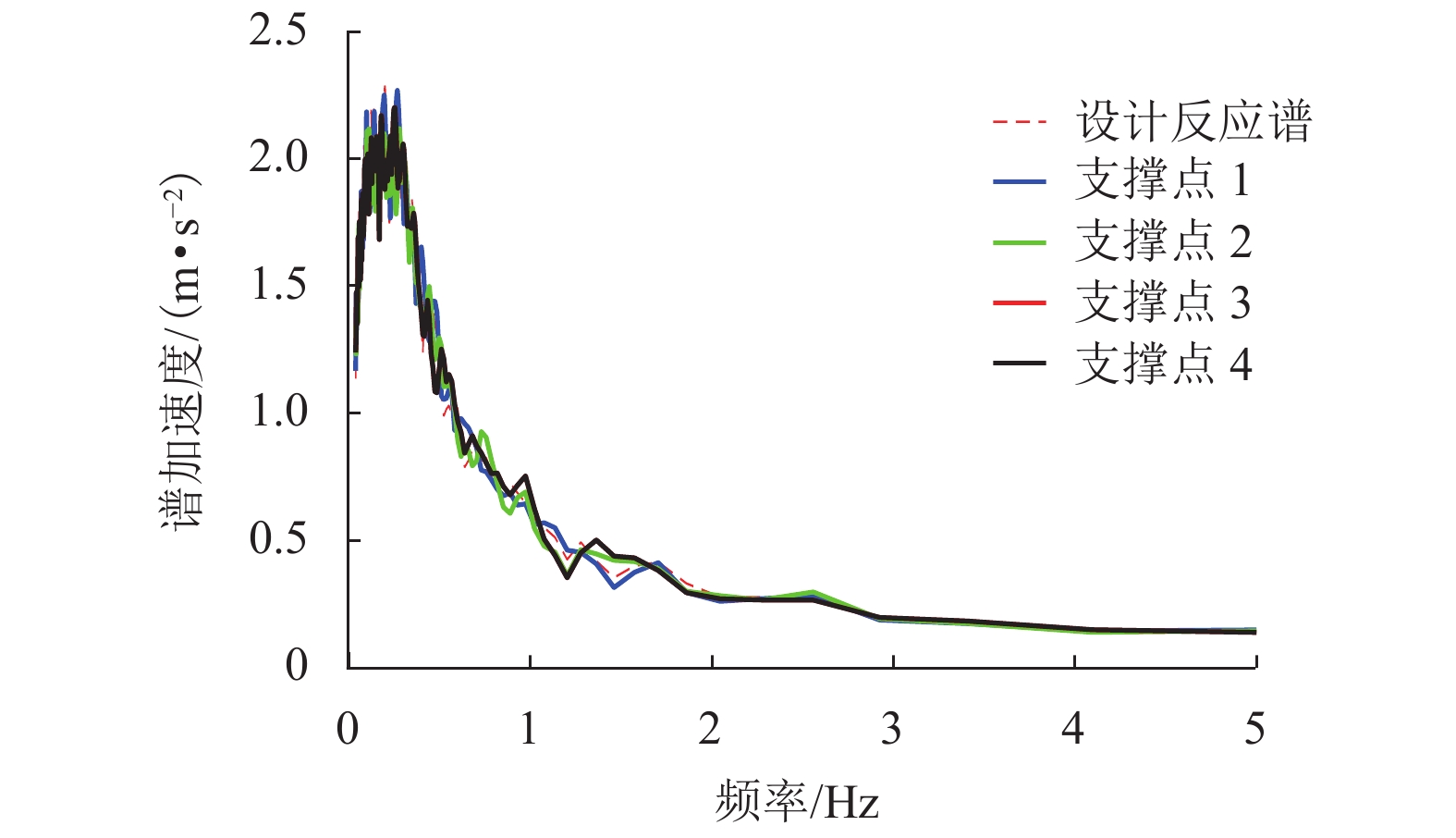
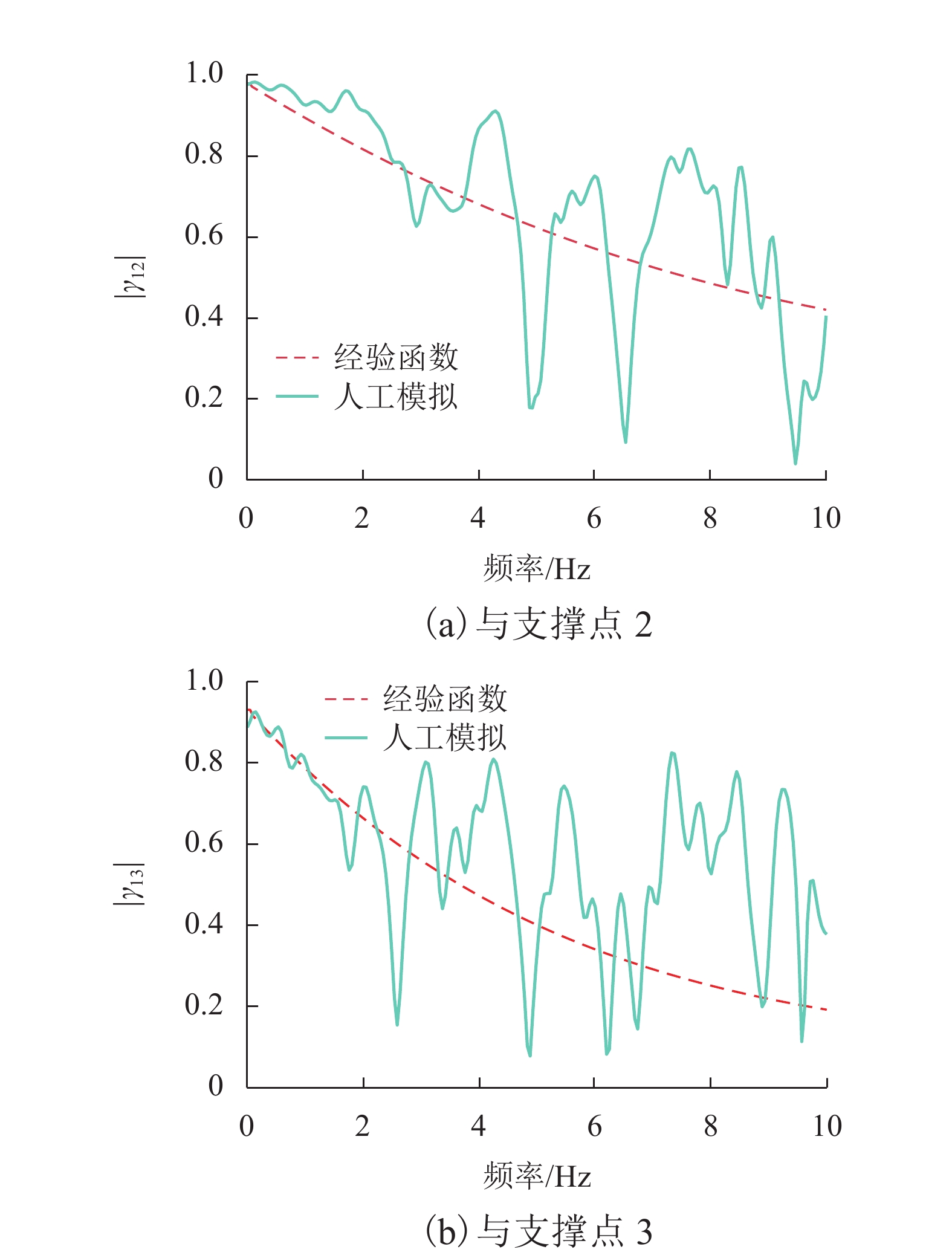
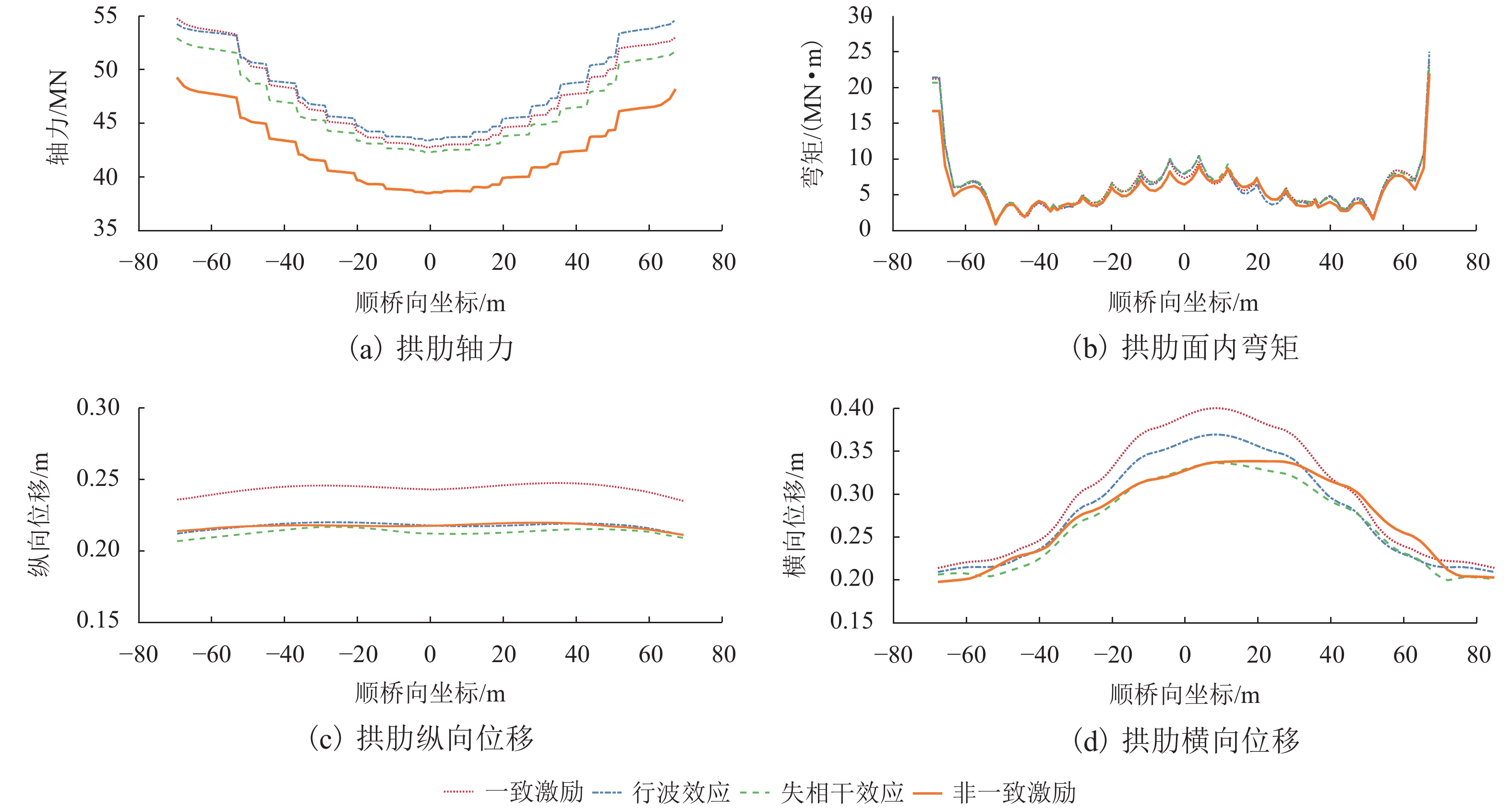
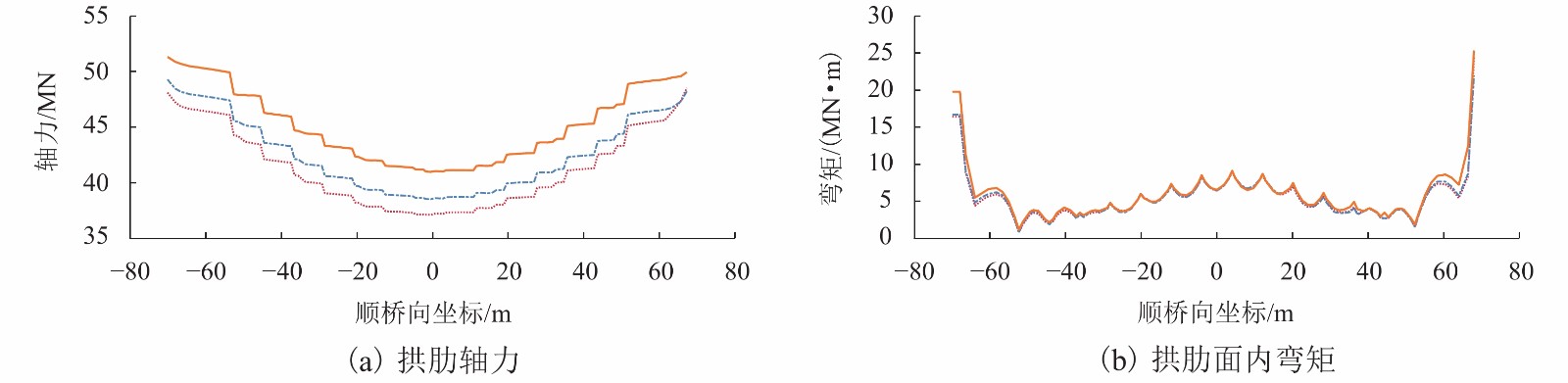
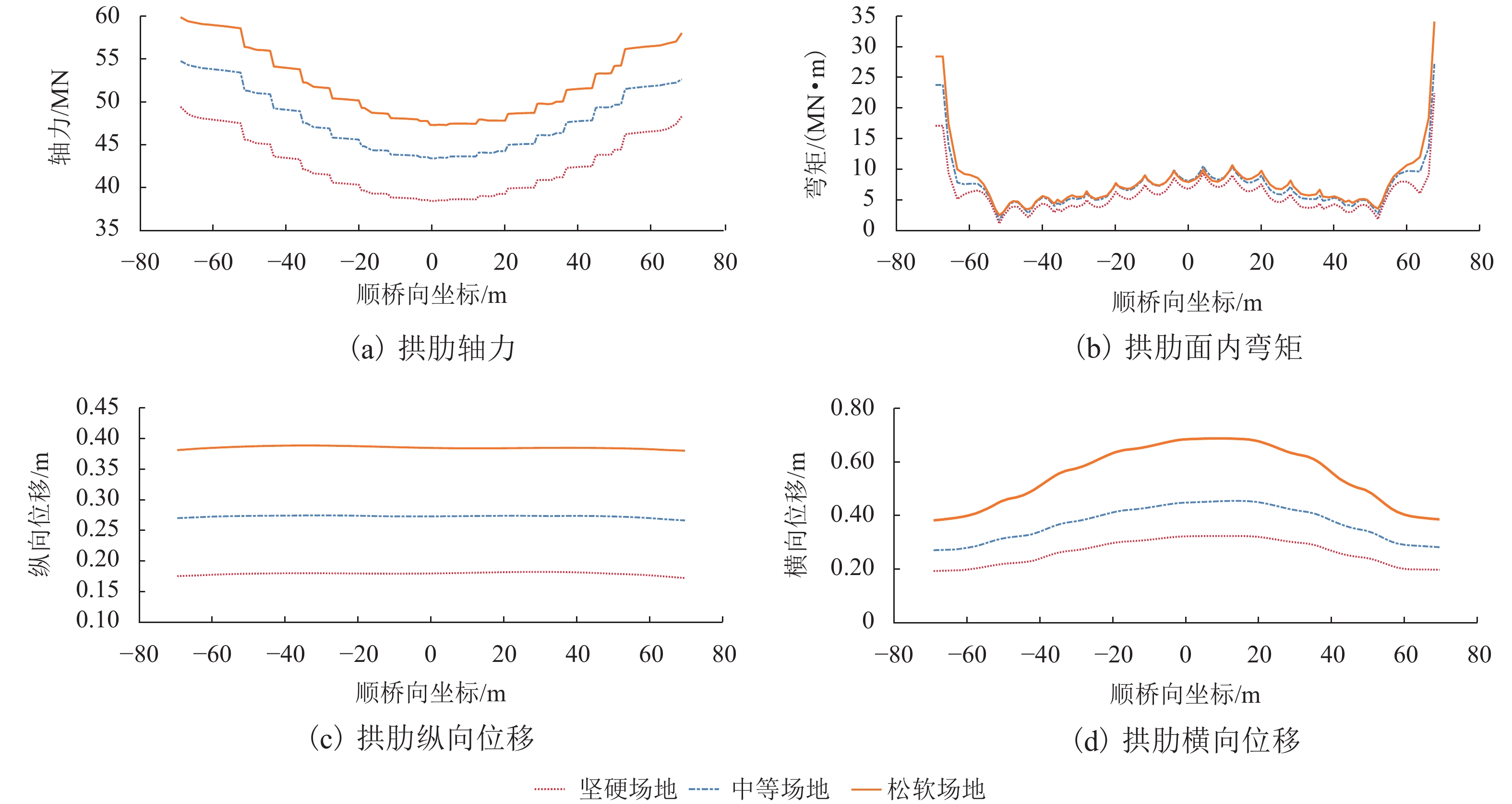
摘要: 为研究多点多维地震动作用下大跨度连续梁拱桥的动力响应，以我国南方某主跨为139 m的钢管混凝土连续梁拱桥为研究对象，基于有限元软件OpenSEES建立桥梁的三维有限元分析模型，人工合成空间非一致地震动，探究地震动的失相干程度、场地条件及行波波速对桥梁动力响应的影响. 研究结果表明：地震波的空间变异性效应会对连续梁拱桥的地震响应产生明显影响，仅考虑一致地震动激励会高估桥梁结构的地震响应；场地效应对桥梁地震响应的影响规律最为突出，随着支撑点处的场地越来越松软，桥梁各个部位的内力及位移响应均大幅增加；地震动的失相干效应越明显，桥梁拱肋的内力越大，位移越小；行波效应对桥梁结构的地震反应没有较为明确的影响规律，但不可忽略其作用，仅考虑行波效应会严重低估下部结构的地震响应；在大跨度桥梁结构的地震响应分析中，应着重考虑地震动的空间变异性效应，并且准确衡量各因素的作用.Abstract: In order to investigate the dynamic responses of a long-span continuous beam-arch bridge subjected to multi-support and multi-dimensional ground motions, a finite element model for a concrete-filled steel tubular arch bridge with a span of 139 m in South China was built using OpenSEES software. The spatially varying ground motions were artificially simulated to investigate the effects of the coherency loss, local site conditions, and wave-passage. The results show that the spatially varying ground motions have significant influence on the dynamic responses of bridge, and considering uniform excitations only might overestimate the responses. The influence of the local site effect on the seismic responses of the bridge is the most prominent. With softer site conditions at the support points, the inner forces and displacements responses of each part of the bridge are greatly increased. Weakly correlated ground motions can lead to larger arch inner forces and smaller arch peak displacements. Although the wave-passage effect has no obvious tendency, its influence is not negligible. Only considering the wave-passage effect may seriously underestimate the seismic responses of the bridge substructure. Therefore, the spatially varying ground motions should be considered in the seismic responses analysis of long-span bridges, and the effect of each factor should be accurately measured.
-
图 3 各支撑点处非一致地震动加速度与位移时程曲线(工况4)
Figure 3. Generated spatially varying ground motions on different sites (case 4)
图 4 人工生成地震波反应谱与设计反应谱对比（坚硬场地）
Figure 4. Comparison of the simulated acceleration and the code response spectrum (firm)
图 5 人工生成地震波失相干损失与经验函数对比
Figure 5. Comparison of the coherency loss between simulated ground motions and the empirical function
图 6 多点多维地震动下桥梁拱肋峰值响应包络图
Figure 6. Peak seismic responses envelope of arch rib under multi-point and multi-dimensional ground motions
图 7 不同失相干程度时拱肋峰值响应包络图
Figure 7. Peak seismic responses envelope of arch rib with different coherency loss
图 8 不同场地类型时拱肋峰值响应包络图
Figure 8. Peak seismic responses envelope of arch rib with different soil conditions
图 9 不同行波波速时拱肋峰值响应包络图
Figure 9. Peak seismic responses envelope of bridge with different apparent wave velocities
表 1 桥梁自振特性
Table 1. Vibration characteristics of bridge
模态阶数 自振周期/s 频率/Hz 振型描述 1 2.00 0.50 拱肋横向振动 2 0.95 1.05 桥梁纵向振动 3 0.92 1.09 拱肋横向反对称振动 4 0.89 1.12 主梁横向对称振动 5 0.58 1.72 桥梁横向振动 
下载: 导出CSV
表 2 空间变异性地震动工况
Table 2. Spatial ground motion cases
工况 场地类型 行波波速/（m•s−1） 相干损失程度 1 FFFF 无穷 无 2 FFFF 500 无 3 FFFF 无穷 中等 4 FFFF 500 中等 5 FMMF 500 中等 6 FSSF 500 中等 7 FFFF 250 中等 8 FFFF 1000 中等 9 FFFF 500 低 10 FFFF 500 高
下载: 导出CSV
表 3 多点多维地震动下桥墩峰值响应
Table 3. Peak seismic responses of pier under multi-point and multi-dimensional ground motions
计算工况 2# 桥墩 3# 桥墩 剪力/MN 弯矩/（MN•m） 位移/m 剪力/MN 弯矩/（MN•m） 位移/m 一致激励 380.641 285.262 0.182 402.244 429.243 0.184 仅行波效应 130.215 124.308 0.168 124.670 377.329 0.164 仅失相干 323.037 202.167 0.173 394.313 418.430 0.168 非一致激励 302.871 173.473 0.171 310.209 357.471 0.175
下载: 导出CSV
表 4 不同失相干程度时桥墩峰值响应
Table 4. Peak seismic responses of pier with different coherency loss
相干程度 2# 桥墩 3# 桥墩 剪力/MN 弯矩/（MN•m） 位移/m 剪力/MN 弯矩/（MN•m） 位移/m 高相干 470.964 298.238 0.170 449.581 377.796 0.177 中等相干 302.871 173.473 0.171 310.209 357.471 0.175 低相干 226.365 153.311 0.174 528.717 366.331 0.168
下载: 导出CSV
表 5 不同场地类型时桥墩峰值响应
Table 5. Peak seismic responses of pier with different soil conditions
场地类型 2# 桥墩 3# 桥墩 剪力/MN 弯矩/（MN•m） 位移/m 剪力/MN 弯矩/（MN•m） 位移/m 坚硬 302.871 173.473 0.171 310.209 357.471 0.175 中等 383.243 241.076 0.254 368.985 411.714 0.260 松软 415.674 237.745 0.349 511.267 437.504 0.360
下载: 导出CSV
表 6 不同行波波速时桥墩峰值响应
Table 6. Peak seismic responses of pier with different apparent wave velocities
行波波速/（m•s−1） 2# 桥墩 3# 桥墩 剪力/MN 弯矩/（MN•m） 位移/m 剪力/MN 弯矩/（MN•m） 位移/m 1000 339.609 268.676 0.172 308.533 420.712 0.174 500 302.871 173.473 0.171 310.209 357.471 0.175 250 270.429 178.490 0.172 252.022 390.461 0.174
下载: 导出CSV
-
夏修身. 铁路连续梁拱组合桥基于摩擦摆支座的减隔震研究[J]. 西北地震学报,2012,34(4): 350-354.XIA Xiushen. Seismic isolation of combined system of continuous girder-arch railway bridge using friction pendulum bearing[J]. Northwestern Seismological Journal, 2012, 34(4): 350-354. ZANARDO G, HAO H, MODENA C. Seismic response of multi-span simply supported bridges to a spatially varying earthquake ground motion[J]. Earthquake Engineering & Structural Dynamics, 2002, 31(6): 1325-1345. 李晰,何澜,李倩,等. 脉冲型地震动对CFST拱桥抗震性能的影响分析[J]. 西南交通大学学报,2019,54(4): 731-740. doi: 10.3969/j.issn.0258-2724.2014.05.001LI Xi, HE Lan, LI Qian, et al. Effect of pule-like ground motion on seismic performance of concrete-filled steel tubular arch bridge[J]. Journal of Southwest Jiaotong University, 2019, 54(4): 731-740. doi: 10.3969/j.issn.0258-2724.2014.05.001 王立宪,刘屺阳,狄生奎,等. 非一致激励下钢管混凝土拱桥平稳随机地震响应分析[J]. 兰州理工大学学报,2016,42(2): 124-129. doi: 10.3969/j.issn.1673-5196.2016.02.025WANG Lixian, LIU Qiyang, DI Shengkui, et al. Analysis of stationary random seismic response of arch bridge with concrete-filled steel tubes under non-uniform excitation[J]. Journal of Lanzhou University of Technology, 2016, 42(2): 124-129. doi: 10.3969/j.issn.1673-5196.2016.02.025 LI B, CHOUW N. Experimental investigation of inelastic bridge response under spatially varying excitations with pounding[J]. Engineering Structures, 2014, 79: 106-116. doi: 10.1016/j.engstruct.2014.08.012 BI K, HAO H, REN W X. Seismic response of a concrete filled steel tubular arch bridge to spatially varying ground motions including local site effect[J]. Advances in Structural Engineering, 2013, 16(10): 1799-1817. doi: 10.1260/1369-4332.16.10.1799 马凯,钟剑,袁万城,等. 非一致地震激励下飘浮体系斜拉桥易损性分析[J]. 同济大学学报 (自然科学版),2017,45(12): 1744-1754.MA Kai, ZHONG Jian, YUAN Wancheng, et al. Fragility analysis of floating cable-stayed bridge under non-uniform seismic excitation[J]. Journal of Tongji University (Natural Science), 2017, 45(12): 1744-1754. HAN L H, YAO G H, ZHAO X L. Tests and calculations for hollow structural steel (HSS) stub columns filled with self-consolidating concrete (SCC)[J]. Journal of Constructional Steel Research, 2005, 61(9): 1241-1269. doi: 10.1016/j.jcsr.2005.01.004 SCOTT B D, PARK R, PRIESTLEY M J N. Stress-strain behavior of concrete confined by overlapping hoops at low and high strain ratio Rates[D]. Lulea: Lulea University of Technology, 1989. MENEGOTTO M. Method of analysis for cyclically loaded RC plane frames including changes in geometry and non-elastic behavior of elements under combined normal force and bending[C]//Resistance and Ultimate Deformability of Structures Acted on by Well Defined Repeated Loads. Lisbon: IABSE, 1973: 15-22. JOYNER W B, BOORE D M. Measurement,characterization,and prediction of strong ground motion[J]. Geotechnical Special Publication, 1988, 6(6): 43-102. 贾宏宇,蓝先林,陈航,等. 基于相位差谱的非平稳地震波合成及应用[J]. 西南交通大学学报,2019,54(3): 453-460.JIA Hongyu, LAN Xianlin, CHEN Hang, et al. Synthesis of non-stationary seismic waves based on phase difference spectrum and its application[J]. Journal of Southwest Jiaotong University, 2019, 54(3): 453-460. BI K, HAO H. Modelling and simulation of spatially varying earthquake ground motions at sites with varying conditions[J]. Probabilistic Engineering Mechanics, 2012, 29: 92-104. doi: 10.1016/j.probengmech.2011.09.002 HAO H, OLIVEIRA C S, PENZIEN J. Multiple-station ground motion processing and simulation based on SMART-1 array data[J]. Nuclear Engineering and Design, 1989, 111(3): 293-310. doi: 10.1016/0029-5493(89)90241-0 ZHAO L, HAO H, BI K, et al. Numerical study of the seismic responses of precast segmental column bridge under spatially varying ground motions[J]. Journal of Bridge Engineering, 2018, 23(12): 04018096.1-04018096.18. -
下载:
点击查看大图
计量
- 文章访问数: 684
- HTML全文浏览量: 329
- PDF下载量: 50
- 被引次数: 0