侧边加劲半圆形波纹钢板墙的抗侧性能

周林丽; 谭平; 滕晓飞

doi:10.3969/j.issn.0258-2724.20190319

侧边加劲半圆形波纹钢板墙的抗侧性能

doi: 10.3969/j.issn.0258-2724.20190319

周林丽^1,2,,
谭平^1,2, ,,
滕晓飞^1,2

基金项目: 国家重点研发计划（2016YFC0701502）；广东省教育厅创新团队项目（2016KCXTD016）；广州市高校“羊城学者”首席科学家（1201541630）

详细信息

作者简介:
周林丽（1984—），女，博士研究生，研究方向为工程结构消能减震，E-mail：zll_1984@e.gzhu.edu.cn

通讯作者:
谭平（1973—），男，研究员，研究方向为结构工程抗震与减震控制，E-mail：ptan@gzhu.edu.cn

中图分类号: TU392.4
计量
- 文章访问数: 467
- HTML全文浏览量: 251
- PDF下载量: 15
- 被引次数: 0
出版历程
- 收稿日期: 2019-04-23
- 修回日期: 2019-07-12
- 网络出版日期: 2020-12-18
- 刊出日期: 2021-06-15

Lateral Performance of Semicircular Corrugated Steel Plate Shear Wall with Edge Stiffeners

ZHOU Linli^{1,2
,},
TAN Ping^{1,2
, ,},
TENG Xiaofei^1,2

摘要

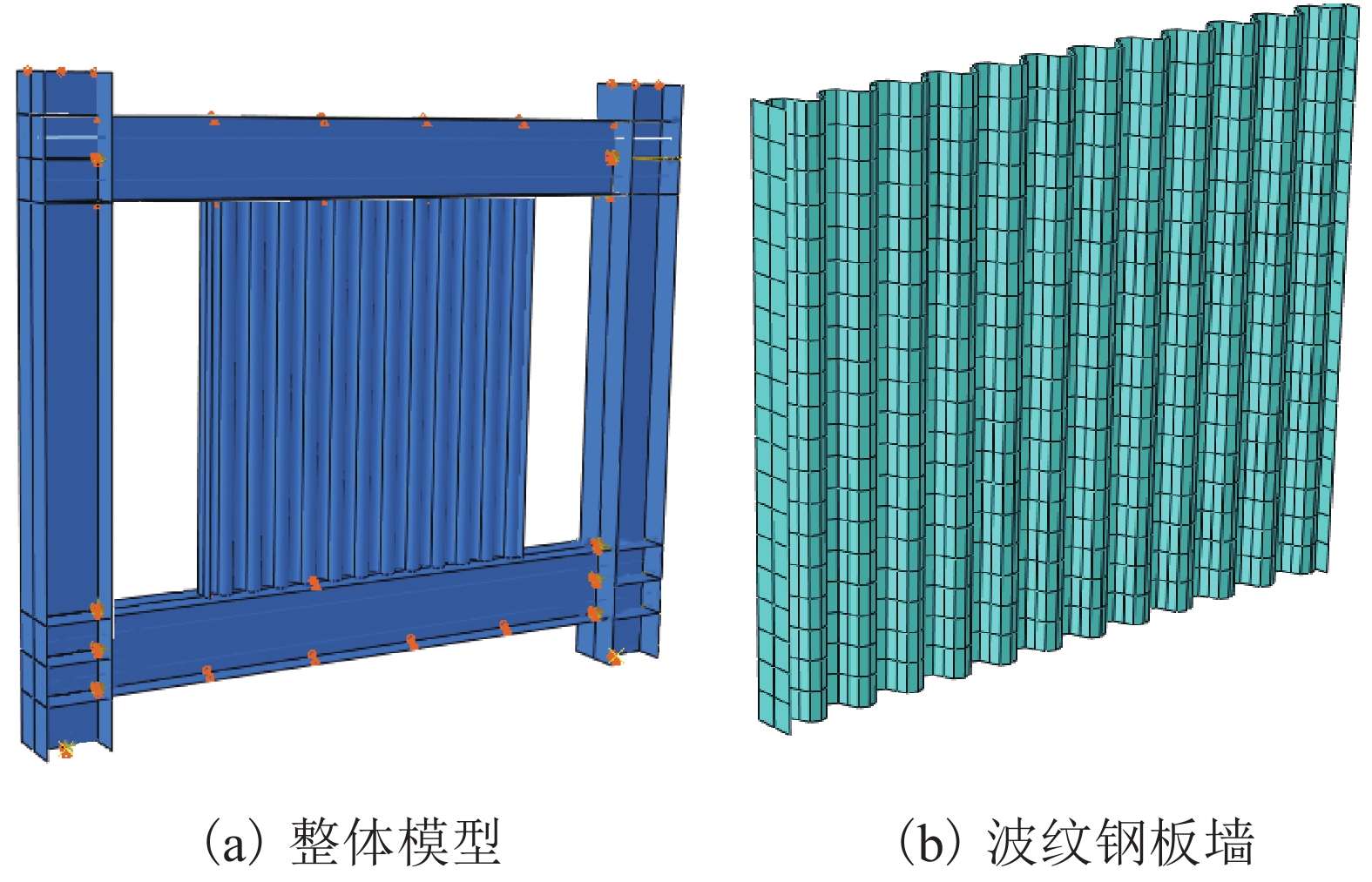
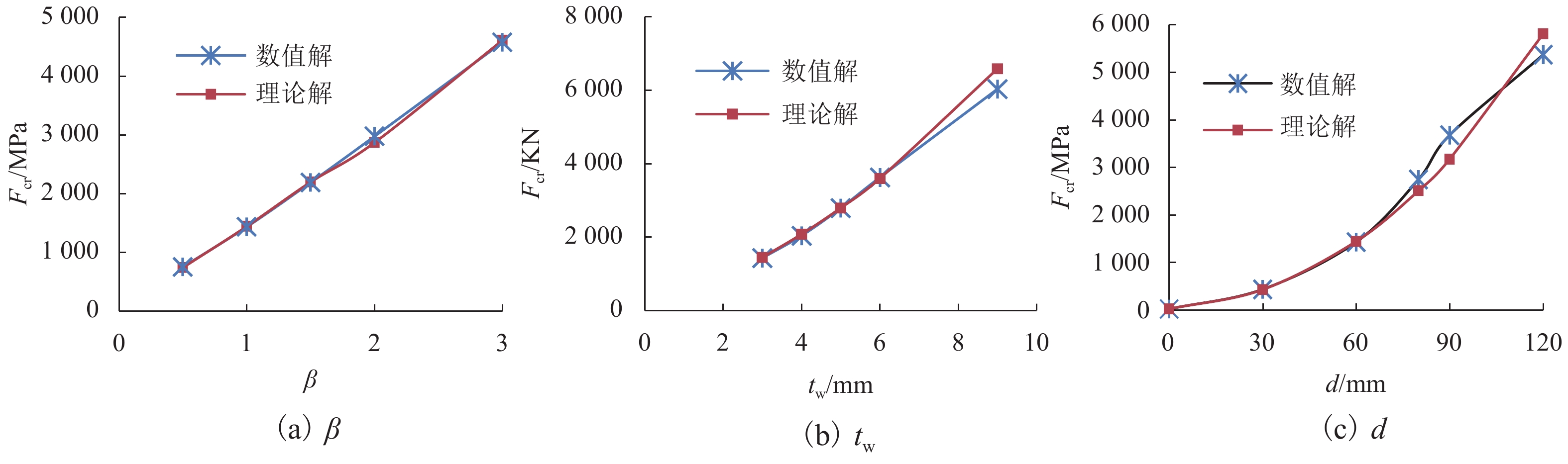
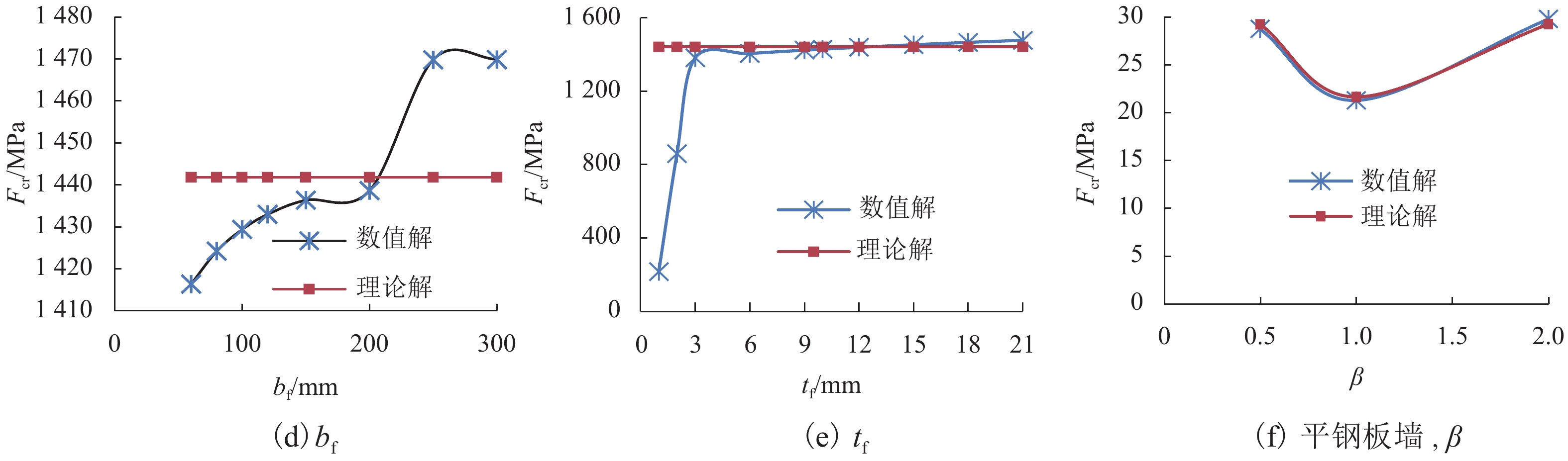
摘要: 为改善平钢板剪力墙受剪易屈曲及面外刚度小的问题，提出侧边加劲半圆形波纹钢板墙. 基于两边连接侧边加劲半圆形波纹钢板墙的力学特点给出其简化力学模型，推导了弹性初始刚度及承载力公式，并给出弹性屈曲临界荷载计算公式；采用有限元软件ABAQUS对22个单层侧边加劲半圆形波纹钢板墙进行了弹性屈曲分析及非线性推覆分析，验证了理论公式的有效性；研究了各设计参数对侧边加劲半圆形波纹钢板墙屈曲性能和破坏模式的影响. 研究结果表明：侧边加劲半圆形波纹钢板墙的弹性屈曲临界荷载较平钢板墙有显著提高；为保证侧边加劲半圆形波纹钢板墙发生整体屈曲，圆形直径及加劲肋厚度比应满足相应取值要求；随着跨高比的增大、高厚比的减小及半圆形直径的增大，钢板墙的弹性屈曲临界荷载基本呈线性增长；侧边加劲肋的肋宽及肋厚对波纹钢板墙弹性屈曲临界荷载的影响较小；在侧向荷载作用下，当直径大于30 mm时，侧边加劲半圆形波纹钢板墙的屈服先于屈曲；侧边加劲半圆形波纹钢板墙存在3种破坏模式，即弯曲破坏、弯剪破坏及形成拉力带形式的“褶皱”.
- 侧边加劲半圆形波纹钢板墙 /
- 屈曲 /
- 抗侧性能 /
- 破坏模式
Abstract: A semicircular corrugated steel plate shear wall with edge stiffeners (CSPSW) was proposed to improve the premature buckling and small out of plane stiffness of flat steel plate shear wall. Theoretical elastic initial stiffness and bearing capacity were derived according to the simplified mechanical model which based on the mechanical characteristics of the CSPSW, and the elastic buckling critical load formula of the proposed steel plate shear wall was also given. Elastic buckling analysis and nonlinear pushover analysis of 22 single-story CSPSW were carried out by using the finite element software ABAQUS, the validity of the theoretical formula was verified. The effects of various design parameters on the buckling performance and failure mode of CSPSW were investigated. The results show that the elastic buckling critical load and bearing capacity of the proposed CSPSW are much higher than the plain steel plate wall. The circular diameter and stiffener thickness ratio should meet the corresponding value requirements to ensure the overall buckling of CSPSW. With the increase of the span height ratio, the decrease of the depth-thickness ratio and the increase of the circular diameter, the elastic buckling critical load of CSPSW increases linearly. The rib width and rib thickness of the side stiffeners have little effect on the elastic buckling critical load of CSPSW. Yielding of the CSPSW prior to buckling under lateral load when the circular diameter is bigger than 30 mm. There are three failure modes of CSPSW, namely bending, bend-shearing, and forming “pleats” in the form of tensile bands.
- semicircular corrugated steel plate shear wall with edge stiffeners /
- buckling /
- lateral performance /
- failure mode

HTML全文

图 1 侧边加劲波纹钢板墙

Figure 1. Schematic diagram of CSPSW

下载: 全尺寸图片幻灯片

图 2 波纹钢板墙简化力学模型

Figure 2. Simplified mechanical model of CSPSW

下载: 全尺寸图片幻灯片

图 3 有限元模型

Figure 3. Finite element model

下载: 全尺寸图片幻灯片

图 4 侧边加劲波纹钢板墙的典型屈曲模态

Figure 4. Typical buckling modes of CSPSW

下载: 全尺寸图片幻灯片

图 5 侧边加劲波纹钢板墙弹性屈曲临界荷载数值解与理论解对比

Figure 5. Numerical solution and theoretical solution comparison of elastic buckling critical load on CSPSW

下载: 全尺寸图片幻灯片

图 6 波纹钢板墙的典型破坏模式

Figure 6. Typical failure modes of CSPSW

下载: 全尺寸图片幻灯片

表 1 理论公式的验证

Table 1. Verification of theoretical formulas

模型编号	屈曲荷载			K₀			V_y			V_u
模型编号	理论/kN	数值/kN	误差/%	理论/ （kN•mm⁻¹）	数值/ （kN•mm⁻¹）	误差/%	理论/kN	数值/kN	误差/%	理论/kN	数值/kN	误差/%
B-0	1441.82	1429.37	−0.86	122.23	122.24	0.01	1107.41	1040.54	−6.03	1262.45	1189.05	−5.81
B-1	739.50	745.45	0.80	44.39	48.10	8.36	504.92	440.35	−12.80	575.61	548.09	−4.78
B-2	2870.40	2973.44	3.59	269.92	271.73	0.67	2222.26	2260.03	1.70	2533.38	2565.13	1.25
B-3	2073.62	2036.57	−1.79	162.97	161.34	−1.00	1476.55	1374.88	−6.90	1683.27	1670.72	−0.75
B-4	2788.90	2784.04	−0.17	203.71	200.25	−1.69	1845.69	1708.74	−7.41	2104.10	2136.10	1.52
B-5	3592.37	3621.79	0.82	244.45	239.05	−2.21	2214.82	2141.63	−3.30	2524.90	2555.10	1.20
B-6	6579.22	6027.50	−8.39	366.68	355.19	−3.13	3322.23	3275.56	−1.40	3787.34	3817.68	0.80
B-7	428.41	434.48	1.42	122.22	122.70	0.39	705.11	638.50	−9.45	803.83	868.27	8.01
B-8	2506.72	2748.35	9.64	122.23	122.18	−0.04	1110.68	1212.49	9.17	1266.18	1353.84	6.92
B-9	3173.56	3679.34	13.75	122.24	122.18	−0.05	1111.55	1199.23	7.90	1267.17	1341.95	5.90
B-10	5805.27	5369.10	−7.51	122.25	122.19	−0.05	1114.81	1035.82	−7.10	1270.88	1337.98	5.27
B-11	1441.82	1416.38	−1.76	122.23	120.85	−1.13	1107.41	1047.36	−5.40	1262.45	1192.03	−5.58
B-12	1441.82	1436.36	−0.38	122.23	123.00	0.63	1107.41	1097.21	−0.92	1262.45	1261.85	−0.04
B-13	1441.82	1438.63	−0.22	122.23	123.24	0.83	1107.41	1019.69	−7.92	1262.45	1166.13	−7.63
B-14	1441.82	1469.80	1.94	122.23	124.14	1.56	1107.41	1072.53	−3.15	1262.45	1226.04	−2.88
B-15	1441.82	1405.58	−2.51	122.23	119.67	−2.10	1107.41	1017.16	−8.15	1262.45	1165.18	−7.70
B-16	1441.82	1423.91	−1.24	122.23	121.65	−0.47	1107.41	1034.52	−6.58	1262.45	1185.14	−6.12
B-17	1441.82	1453.72	0.83	122.23	124.91	2.19	1107.41	1069.83	−3.40	1262.45	1238.90	−1.87
B-18	1441.82	1466.48	1.71	122.23	126.33	3.35	1107.41	1085.26	−2.00	1262.45	1352.11	7.10
B-19	29.22	28.72	−1.71	−	58.38	−	−	491.46	−	−	630.37	−
B-20	21.62	21.26	1.69	−	177.00	−	−	1141.69	−	−	1322.23	−
B-21	29.24	29.79	1.88	−	395.87	−	−	1656.52	−	−	1892.67	−
注：“−”表示不考虑平钢板剪力墙对应的理论值.

下载: 导出CSV

参考文献(21)

张彬彬,曹万林,潘毅,等. 双向单排配筋T形剪力墙抗震性能试验研究[J]. 土木建筑与环境工程,2011,33(增刊1): 203-208.

ZHANG Binbin, CAO Wanlin, PAN Yi, et al. Experimental study on seismic performance of T-shear wall structure with bidirectional single row of steel bars[J]. Journal of Civil Architectural and Environmental Engineering, 2011, 33(S1): 203-208.

张彬彬,曹万林,张建伟,等. 双向单排配筋Z形剪力墙抗震性能试验研究[J]. 工程抗震与加固改造,2011,33(5): 45-52. doi: 10.3969/j.issn.1002-8412.2011.05.008

ZHANG Binbin, CAO Wanlin, ZHANG Jianwei, et al. Experimental study on seismic performance of Z-shear wall structure with bidirectional single row of steel bars[J]. Earthquake Resistant Engineering and Retrofitting, 2011, 33(5): 45-52. doi: 10.3969/j.issn.1002-8412.2011.05.008

American Institute of Steel Construction. Specification for structural steel buildings: ANSI/AISC360-10[S]. Chicago: [s.n.], 2010.

BERMAN J, BRUNEAU M. Plastic analysis and design of steel plate shear walls[J]. Journal of structural Engineering, 2003, 129(11): 1448-1456.

张哲,李国强,孙飞飞. 波纹腹板钢梁受弯稳定性研究[J]. 工程力学,2011,28(8): 77-82.

ZHANG Zhe, LI Guoqiang, SUN Feifei. Research on flexural stability of the H-beams with corrugated webs[J]. Engineering Mechanics, 2011, 28(8): 77-82.

李国强,范昕,孙飞飞,等. 波纹腹板H型钢梁柱铰接节点静力性能试验研究及有限元分析[J]. 建筑结构学报,2013,34(4): 107-114.

LI Guoqiang, FAN xin, SUN Feifei, et al. Investigation of static behavior of pinned connections of H-beams with corrugated webs[J]. Journal of Building Structures, 2013, 34(4): 107-114.

清华大学, 巴特勒（上海）有限公司. 波浪腹板钢结构应用技术规程: CECS290—2011[S]. 北京: 中国计划出版社, 2011.

中国建筑标准设计研究院有限公司. 门式刚架轻型房屋钢结构技术规范: GB 51022—2015[S]. 北京: 中国建筑工业出版社, 2015.

BOTROS R B G. Nonlinear finite element analysis of corrugated steel plate shear walls[R]. Calgary: University of Calgary, 2006.

兰银娟. 折板钢板剪力墙抗侧力结构理论研究[D]. 西安: 西安建筑科技大学, 2006.

李峰,薛港,问晓朋,等. 斜置单层折板钢板剪力墙的屈曲分析[J]. 水利与建筑工程学报,2011,9(4): 89-92. doi: 10.3969/j.issn.1672-1144.2011.04.021

LI Feng, XUE Gang, WEN Xiaopeng, et al. Elastic buckling analysis for inclined and single fold plate steel shear wall[J]. Journal of Water Resources and Architectural Engineering, 2011, 9(4): 89-92. doi: 10.3969/j.issn.1672-1144.2011.04.021

EMAMI F, MOFID M, VAFAI A. Experimental study on cyclic behavior of trapezoidally corrugated steel shear walls[J]. Engineering Structures, 2013, 48: 750-762.

魏瑶,谭平,李洋,等. 侧边加劲波纹钢板墙弹性剪切屈曲分析[J]. 地震工程与工程振动,2015,35(3): 199-209.

WEI Yao, TAN Ping, LI Yang, et al. Elastic shear buckling analysis of corrugated steel plate wall with edge stiffeners[J]. Earthquake Engineering and Engineering Dynamics, 2015, 35(3): 199-209.

谭平,魏瑶,李洋,等. 波纹钢板剪力墙抗震性能试验研究[J]. 土木工程学报,2018,51(5): 8-15.

TAN Ping, WEI Yao, LI Yang, et al. Experimental investigation on performance of corrugated steel plate shear wall[J]. China Civil Engineering Journal, 2018, 51(5): 8-15.

孙军浩. 波纹钢板剪力墙的抗侧及抗震性能研究[D]. 天津: 天津大学, 2016.

赵秋红,李楠,孙军浩. 波纹钢板剪力墙结构的抗侧性能分析[J]. 天津大学学报(自然科学与工程技术版),2016,49(增刊1): 152-160.

ZHAO Qiuhong, LI Nan, SUN Junhao. Analysis on lateral performance of sinusoidally corrugated steel plate shear walls[J]. Journal of Tianjin University (Science and Technology), 2016, 49(S1): 152-160.

王威,张龙旭,苏三庆,等. 波形钢板剪力墙抗震性能试验研究[J]. 建筑结构学报,2018,39(5): 36-44.

WANG Wei, ZHANG Longxu, SU Sanqing, et al. Experimental research on seismic behavior of corrugated steel plate shear wall[J]. Journal of Building Structures, 2018, 39(5): 36-44.

郭彦林, 童精中, 姜子钦. 波形腹板钢结构设计原理与应用[M]. 北京: 科学出版社, 2015: 15-34.

龙驭球, 包世华. 结构力学[M]. 北京: 高等教育出版社, 2001: 10-26.

孙训方, 方孝淑, 关来泰. 材料力学[M]. 5版. 北京: 高等教育出版社, 2009: 5-21.

哈尔滨工业大学. 钢板剪力墙技术规程: JGJ/T 380—2015[S]. 北京: 中国建筑工业出版社, 2016.

施引文献

附加材料(0)

访问统计