Propagation Effect of Passive Flexible Protection System on Rockfall Impact
-
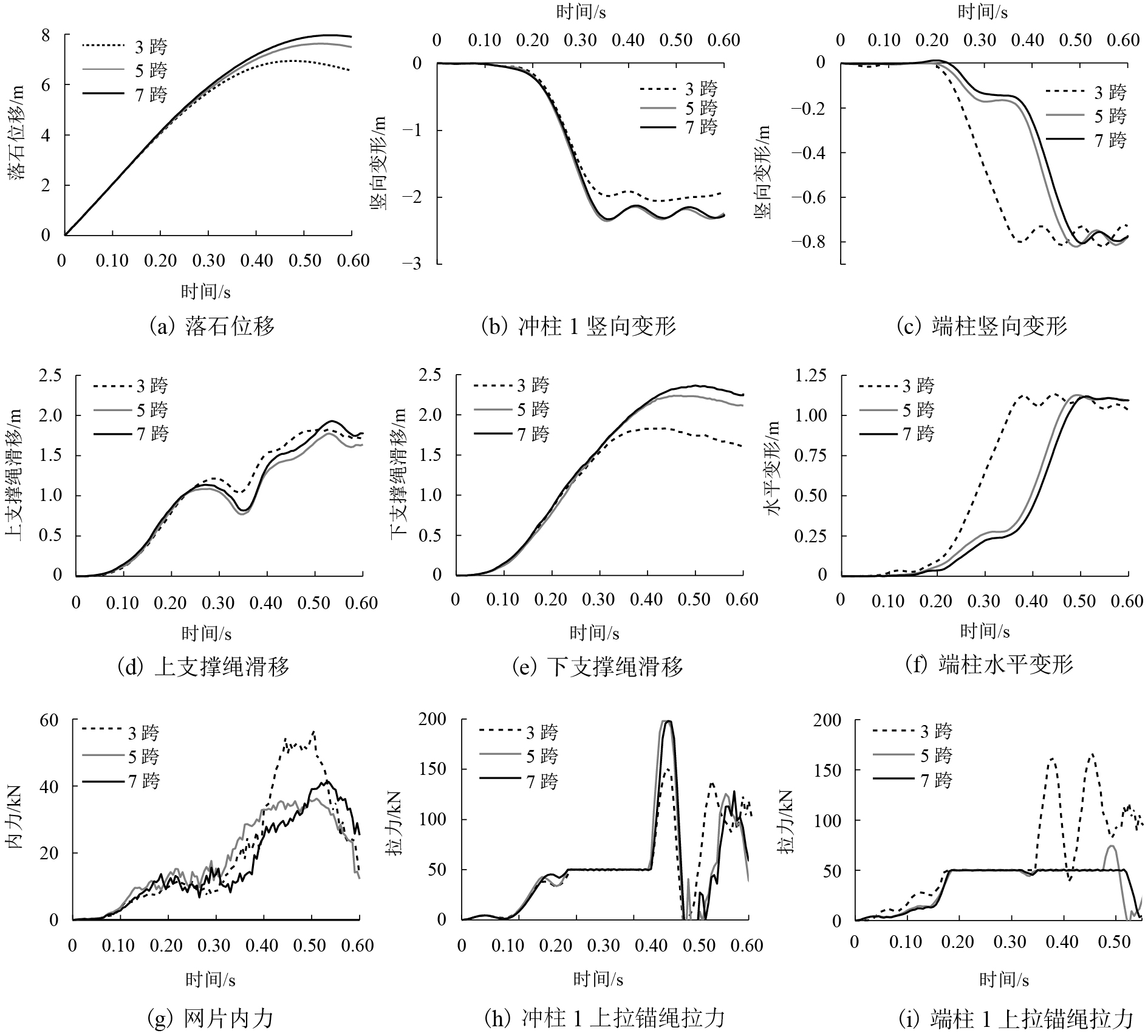
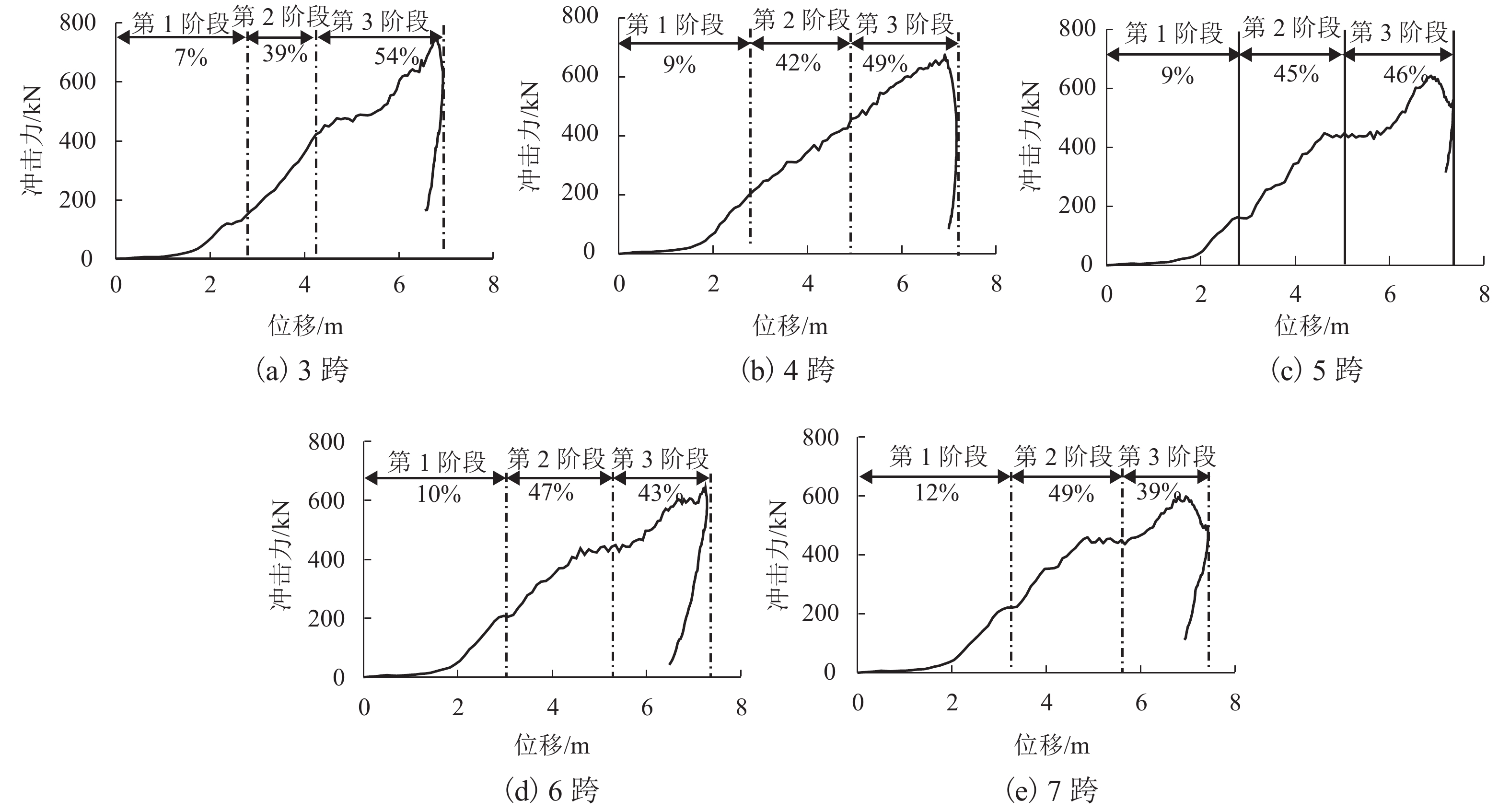
摘要: 被动柔性防护系统遭受落石冲击作用时的波传效应对系统耗能效率存在重要影响，对此开展了能量为1 500 kJ的3跨足尺模型冲击试验，结合LS-DYNA开展了非线性动力分析，对比研究了柔性防护系统的冲击变形、特征点位移和钢丝绳拉力等结构响应沿支撑绳纵向的传播特征. 进一步开展了4跨、5跨、6跨和7跨模型的冲击动力学模拟试验，研究了纵向传播距离增加对冲击响应的影响，包括支撑绳拉力、网片内力和系统冲击力随随波传距离增加的衰减率、构件耗能率和冲击历程的多阶段耗能率变化. 研究结果表明：冲击跨支撑绳拉力明显高于边跨；冲击跨网片内力最大，两侧网片内力急剧衰减，呈高斯型衰减分布；随着系统跨数增加，落石冲击变形极值变化较小，但变形时程变化差异较大；内力衰减变化明显，7跨模型中，支撑绳内力衰减超过了50%，网片内力衰减了40%，冲击力衰减了14%；随着系统传播距离的增加，网片、减压环以及钢柱的耗能减少，钢丝绳和其他摩擦耗能增加，第1、2阶段耗能增大，第3阶段耗能减小.Abstract: The propagation effect of passive flexible protective system under rockfall impact has an important influence on the energy consumption efficiency of the system, a full-scaled impact test, in which the test model had 3 spans in length, was conducted under a kinetic energy of 1 500 kJ. Based on LS-DYNA, the nonlinear numerical simulation on the whole process of the collision and interception was carried out. The comparative analysis on the system deformation, the displacement of characteristic points and tension in the cables between experimental and numerical results was studied. In particular, the propagation effect of the impact load along the longitudinal direction was investigated. Then the numerical models with 4, 5, 6, and 7 spans were calculated respectively to explore the influence of the number of spans on the ultimate system deformation. The attenuation percentage was introduced to discuss the reduction in the internal forces of the cables and wire-ring net, as well as the impact force according to the increase of span number. An energy dissipation ratio was defined, and the ratios distribute among each component and within the three stages as the span number change were analyzed. The results show that the internal force of the support cable is higher than that of the lateral span significantly. The peak internal force of the wire-ring net appears in the impact area, and the internal forces of the net on both lateral sides are very small, so that it presents a Gauss attenuation distribution. With increase of the span number, the maximum elongation of the system changes little, but the time history values have evident differences. The internal force and the impact force decrease obviously, in the 7-span model, the lower support cable tension, the peak internal force of the wire-ring net, and the impact force reduced by 50%, 40%, and 14%, respectively. With the increase of propagation distance of the impact load due to the bigger length of system, the energy dissipation of the wire ring net, the brake rings and the steel column is reduced, and the energy consumption of cables and other components is increased, the energy dissipation ratio increases within the first and second mechanical stages, and decreases within the third stage.
-
Key words:
- flexible rockfall barrier /
- full-scaled test /
- numerical analysis /
- transmission effect
-
图 6 多跨模型特征点及内力时程
Figure 6. Time history of feature points and internal force of mult-span models
表 1 试验模型参数
Table 1. Parameters of test model
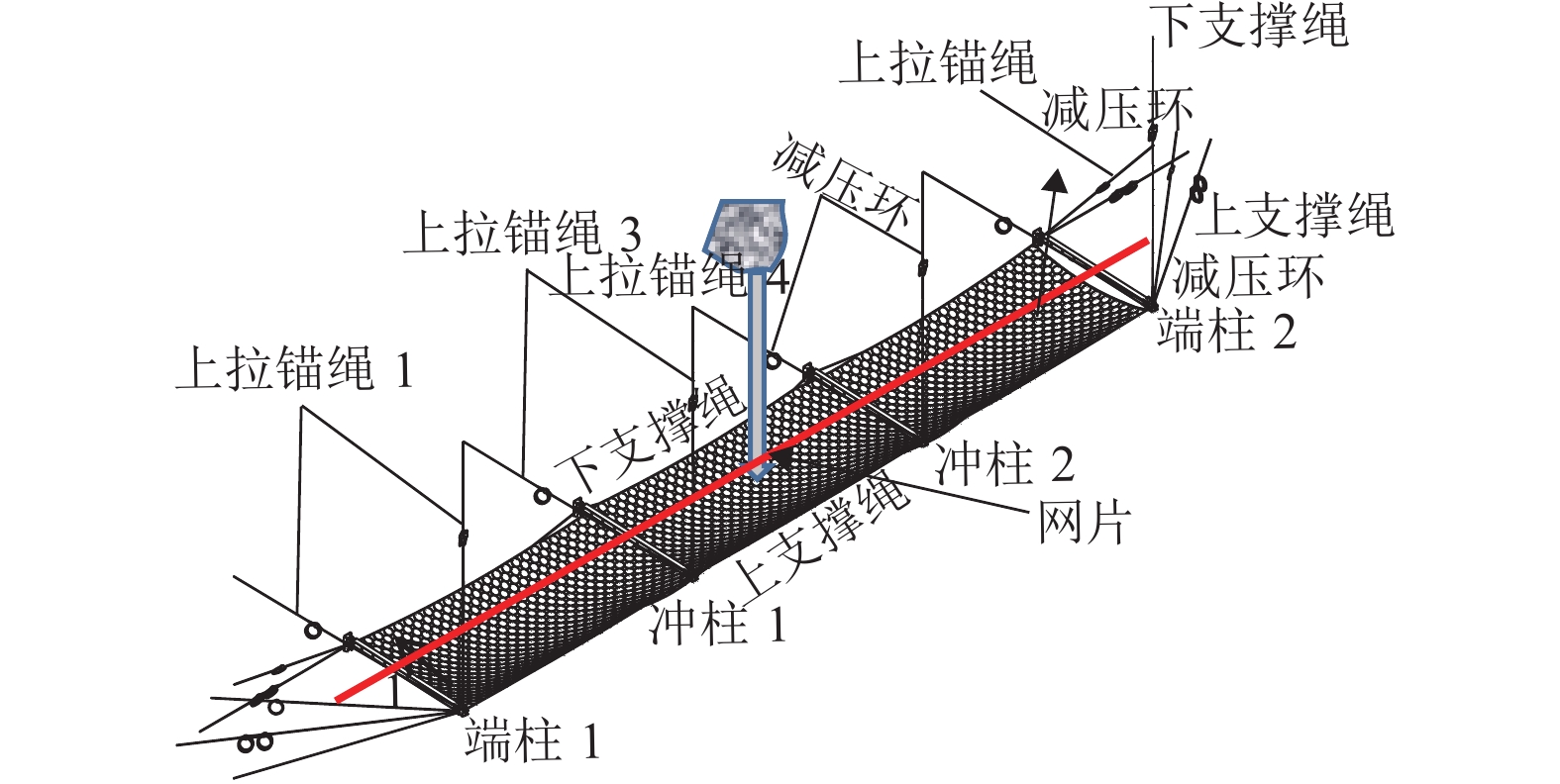
构件名称 型号 材料 环形网 R12/3/300 高强钢丝 上/下支撑主绳 2ϕ20 6 × 19W+FC 上拉锚绳 2ϕ18 6 × 19W+FC 钢柱 HW200 × 200 × 8 × 12 Q235 减压环 GS-8002 圆钢管 
下载: 导出CSV
表 2 有限元模型参数
Table 2. Parameters of finite element model
项目 密度/（kg•m−3） 泊松比 弹性模量/GPa 单元类型 环形网 7 900 0.3 120 梁 钢丝绳 7 900 0.3 150 索 钢柱 7 900 0.3 210 梁 落石 3 000 0.2 20 刚体
下载: 导出CSV
表 3 特征点位移
Table 3. Displacement of feature points
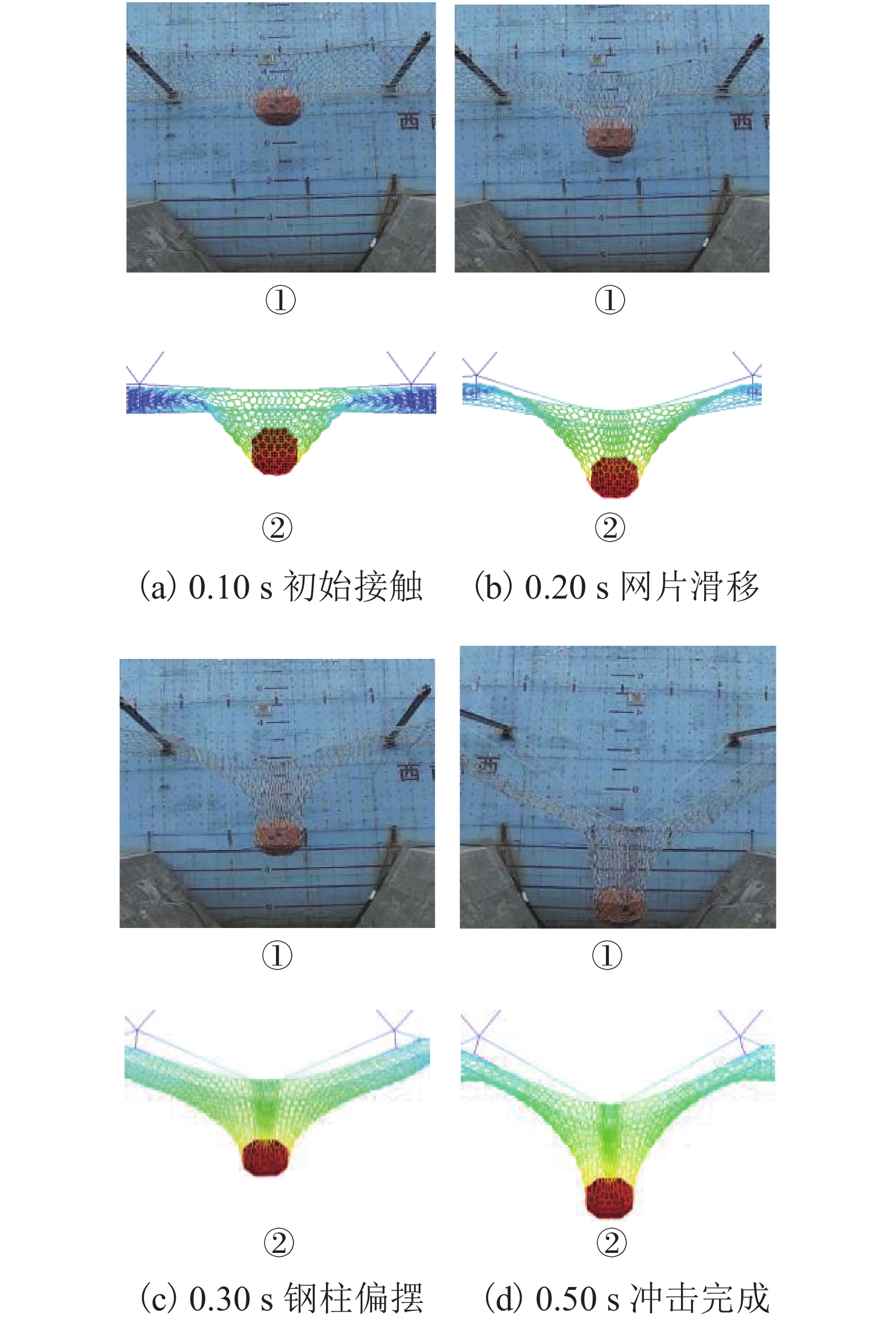
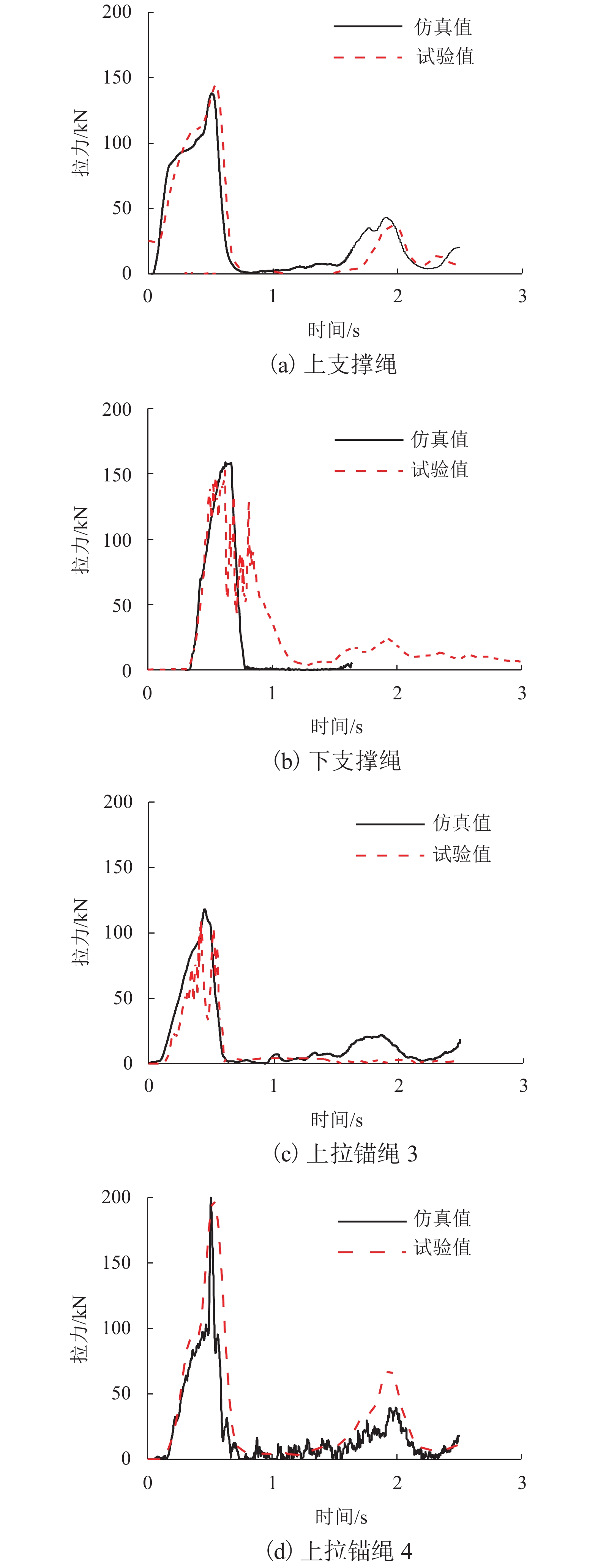
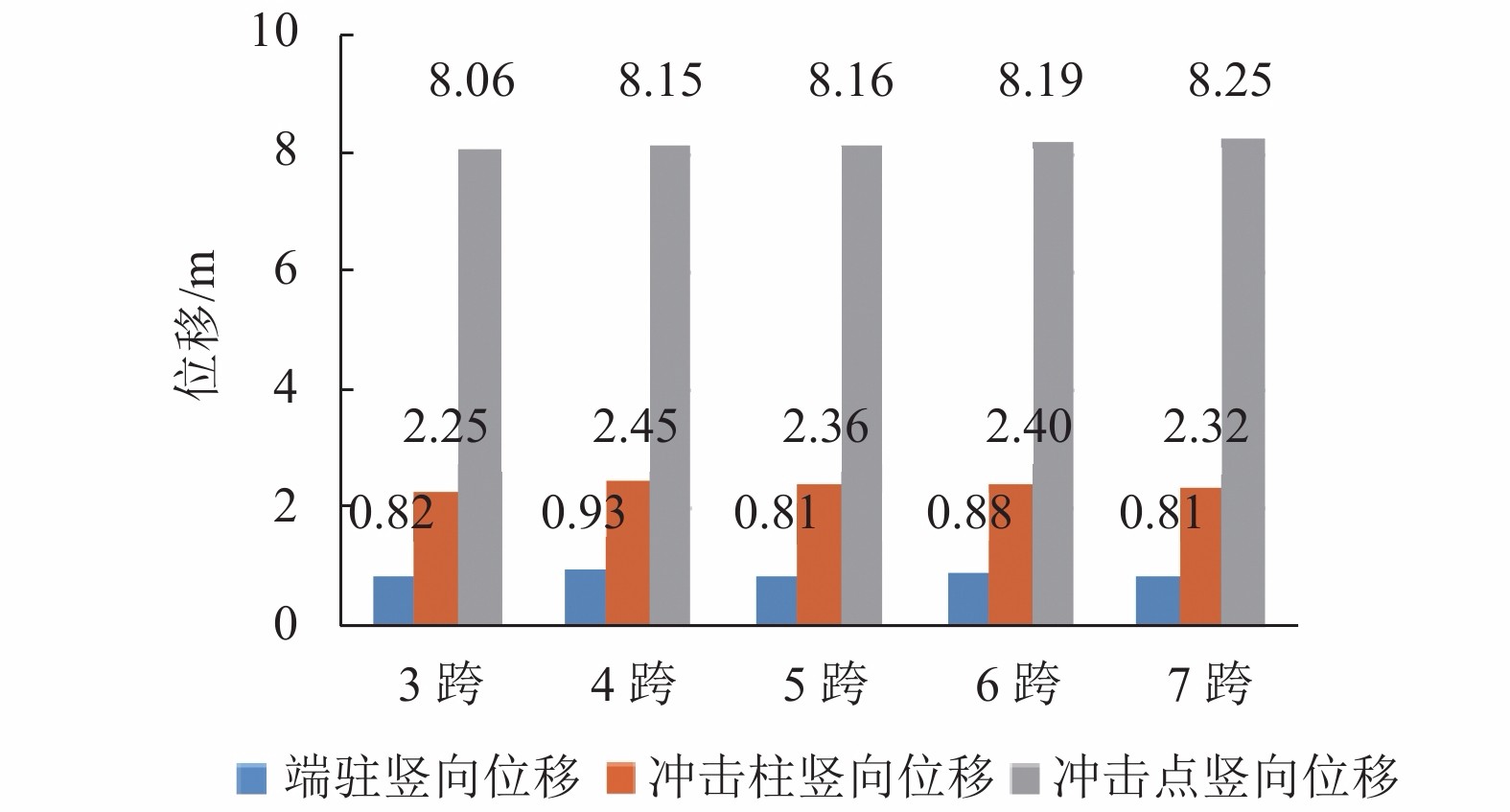
m 位置 试验值 数值仿真值 冲击点 6.22 6.43 端柱 1 自由端 0.67 0.81 冲柱 1 自由端 1.20 1.83 冲柱 2 自由端 1.40 1.83 端柱 2 自由端 0.55 0.81
下载: 导出CSV
表 4 内力值及内力衰减比率
Table 4. Internal force and internal force attenuation percentage
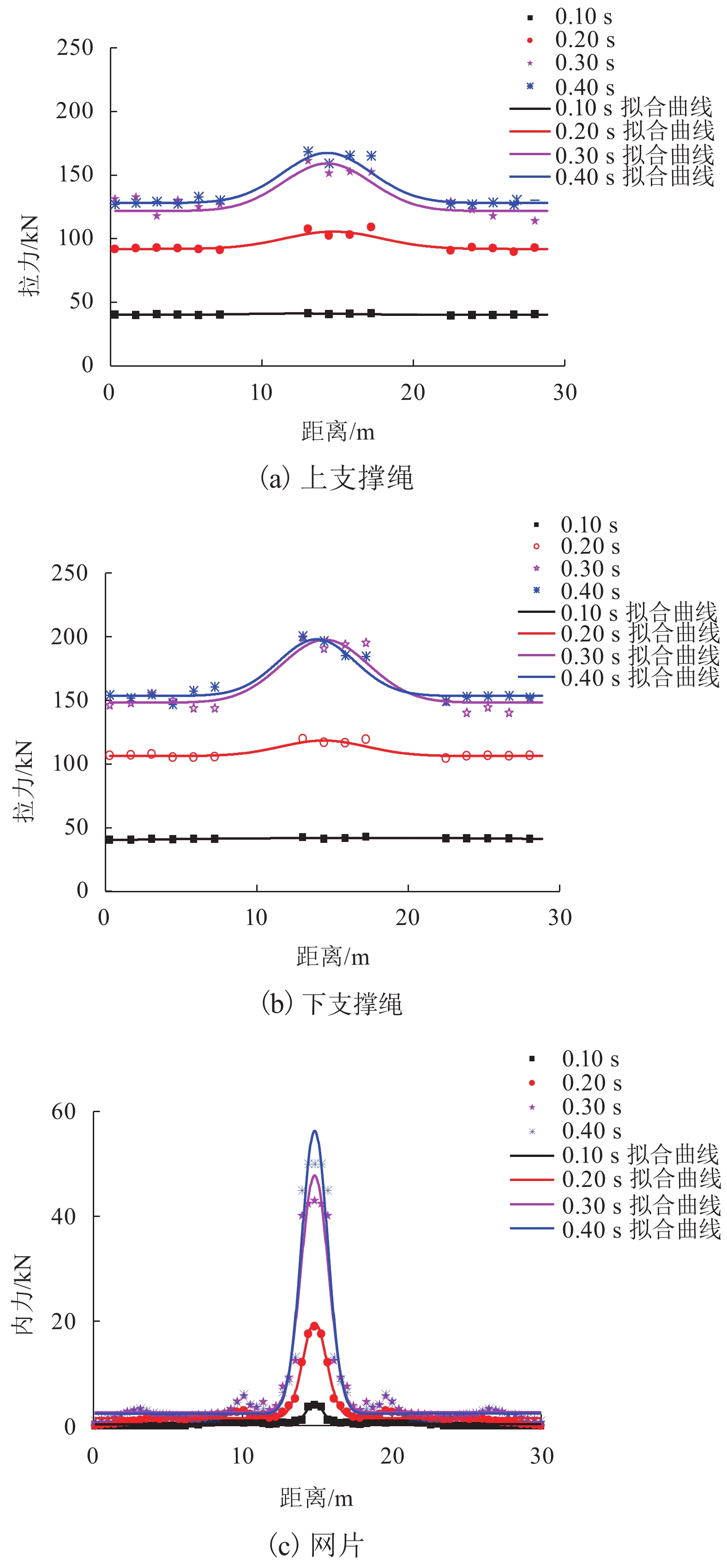
跨数 上支撑绳 下支撑绳 网片内力 冲击力 Fimp/kN Fend/kN ρ/% Fimp/kN Fend/kN ρ/% Fn-span/kN γ/% Fn-span/kN γ/% 3 跨 170.46 133.97 21.40 204.44 155.05 24.15 49.40 0.00 684.15 0.00 4 跨 179.92 110.12 38.80 203.96 153.57 24.71 43.67 11.59 651.77 4.73 5 跨 190.73 100.00 47.57 214.64 112.83 47.43 30.21 38.85 616.25 9.92 6 跨 195.42 100.00 48.83 217.01 100.00 53.92 30.14 38.98 613.08 10.39 7 跨 198.01 100.00 49.50 219.67 100.00 54.48 29.96 39.35 585.65 14.40
下载: 导出CSV
表 5 各构件耗能及耗能比率
Table 5. Energy consumption and energy consumption ratio of each component
跨数 网片 减压环 钢丝绳 钢柱 其他 耗能/kJ 耗能比例/% 耗能/kJ 耗能比例/% 耗能/kJ 耗能比例/% 耗能/kJ 耗能比例/% 耗能/kJ 耗能比例/% 3 跨 392 26.13 943 62.87 45 3.00 120 8.00 42 2.80 4 跨 298 19.87 971 64.73 56 3.73 110 7.33 65 4.33 5 跨 282 18.80 970 64.67 64 4.27 105 7.00 79 5.27 6 跨 282 18.80 945 63.00 75 5.00 103 6.87 95 6.33 7 跨 280 18.67 917 61.13 81 5.40 100 6.67 122 8.13
下载: 导出CSV
-
中华人民共和国铁道部. 铁路沿线斜坡柔性安全防护网: TB/T 3089—2004[S]. 北京: 中国铁道出版社, 2004. 姚建伟, 王瑞琦. SNS柔性防护系统在危岩落石防护中的应用[J]. 华中科技大学学报（城市科学版）, 2006（增刊1）: 46-47, 50.YAO Jianwei, WANG Ruiqi. Application of SNS flexible protection systems for holding rockfall[J]. Journal of HUST（Urban Science Edition）, 2006（S1）: 46-47, 50 梁博. 边坡柔性防护网系统在既有铁路危岩落石中的应用[J]. 华东公路,2015(2): 88-90. European Organisation for Technical Approvals. ETAG 27: guideline for European technical approval of falling rock protection kits[M]. [S.l.]: European Organisation for Technical Approvals, 2008: 13-22 中华人民共和国铁道部. 铁路边坡柔性被动防护产品落石冲击试验方法与评价: TB/T 3449—2016[S]. 北京: 中国铁道出版社, 2017. GENTILINI C, GOVONI L, MIRANDA S D, et al. Three-dimensional numerical modelling of falling rock protection barriers[J]. Computers and Geotechnics, 2012, 44: 58-72. doi: 10.1016/j.compgeo.2012.03.011 GATTARTI G, GOVONI L. Full-scale modelling of falling rock protection barriers[J]. Rock Mechanics and Rock Engineering, 2010,43(3): 261-274. MOON T, OH J, MUN B. Practical design of rockfall catchfence at urban area from a numerical analysis approach[J]. Engineering Geology, 2014, 172: 41-56. doi: 10.1016/j.enggeo.2014.01.004 赵世春,余志祥,韦韬,等. 被动柔性防护网受力机理试验研究与数值[J]. 土木工程学报,2013,46(5): 122-128.ZHAO Shichun, YU Zhixiang, WEI Tao, et al. Test study of force mechanism and numerical calculation of safety netting system[J]. China Civil Engineering Journal, 2013, 46(5): 122-128. YU Z X, QIAO Y K, ZHAO L, et.al. A simple analytical method for evaluation of flexible rockfall barrier part 1:working mechanism and analytical solution[J]. Advanced Steel Construction, 2018, 14(2): 115-141. YU Z X, QIAO Y K, ZHAO L, et al. A simple analytical method for evaluation of flexible rockfall barrier part 2:application and full-scale test[J]. Advanced Steel Construction, 2018, 14(2): 142-165. QI X, YU Z X, ZHAO L, et al. A New numerical modelling approach for flexible rockfall protection barriers based on failure modes[J]. Advanced Steel Construction, 2018, 14(3): 479-495. XU H, GENTILINI C, YU Z, et al. An energy allocation based design approach for flexible rockfall protection barriers[J]. Engineering Structures, 2018, 173(15): 831-852. doi: 10.1016/j.engstruct.2018.07.018 赵世春,余志祥,赵雷,等. 被动防护网系统强冲击作用下的传力破坏机制[J]. 工程力学,2016,33(10): 24-34. doi: 10.6052/j.issn.1000-4750.2016.06.ST08ZHAO Shichun, YU Zhixiang, ZHAO Lei, et al. Damage mechanism of rockfall barriers under strong impact loading[J]. Engineering Mechanics, 2016, 33(10): 24-34. doi: 10.6052/j.issn.1000-4750.2016.06.ST08 徐宏,曹义华,李栋. 先拉伞绳法数学模型及拉直力预测[J]. 航空动力学报,2008,23(4): 706-711.XU Hong, CAO Yihua, LI Dong. Mathematical model and snatch force prediction for the lines-first parachute deployment[J]. Journal of Aerospace Power, 2008, 23(4): 706-711. R. 克拉夫, J. 彭津. 结构动力学[M]. 王光远等, 译校. 北京: 高等教育出版社, 2006: 140-162 -
下载:
点击查看大图
图(7) / 表(5)
计量
- 文章访问数: 686
- HTML全文浏览量: 451
- PDF下载量: 34
- 被引次数: 0