3D Seismic Vulnerability Analysis of Bridge Structural Components Based on Reliability
-
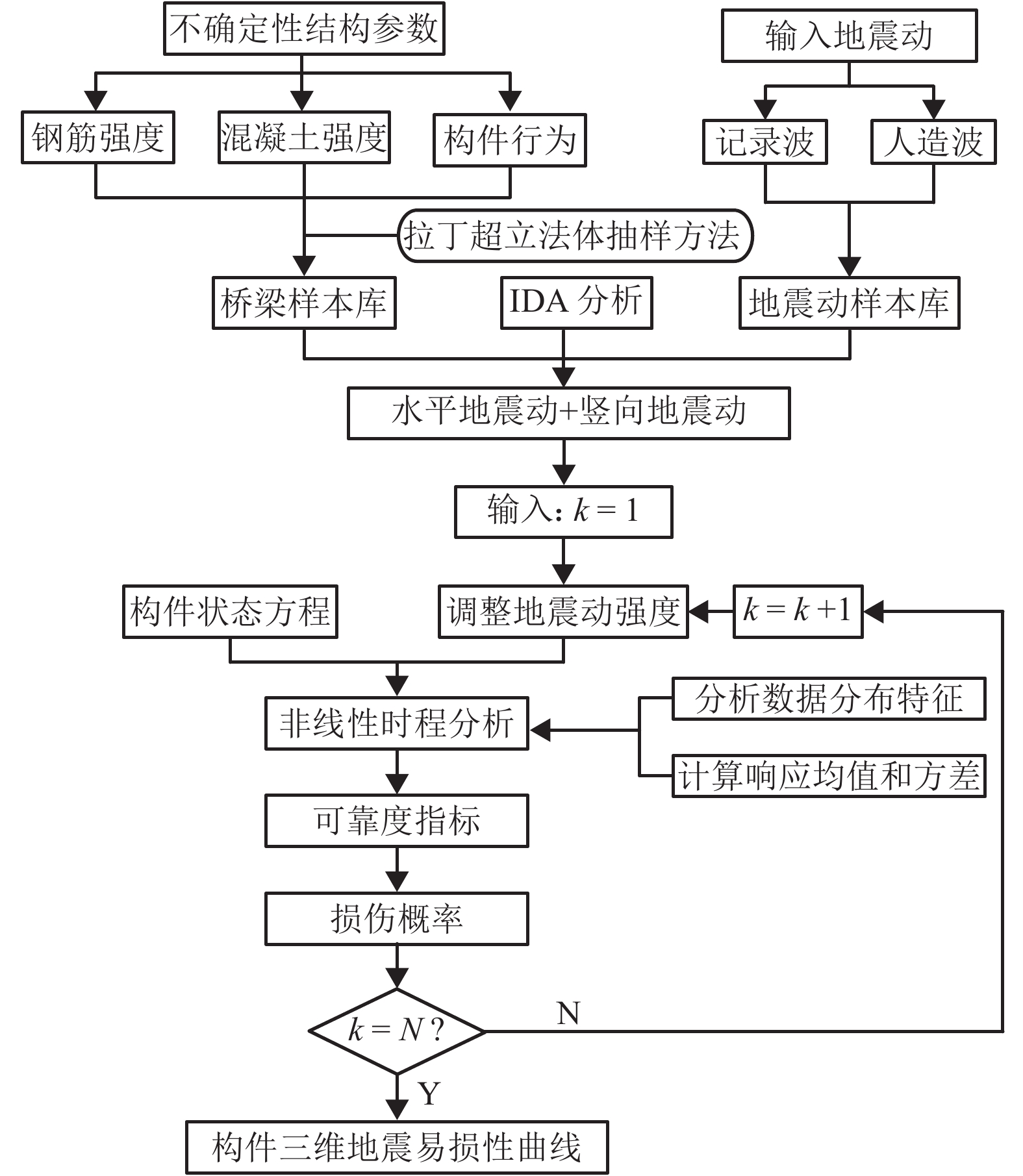
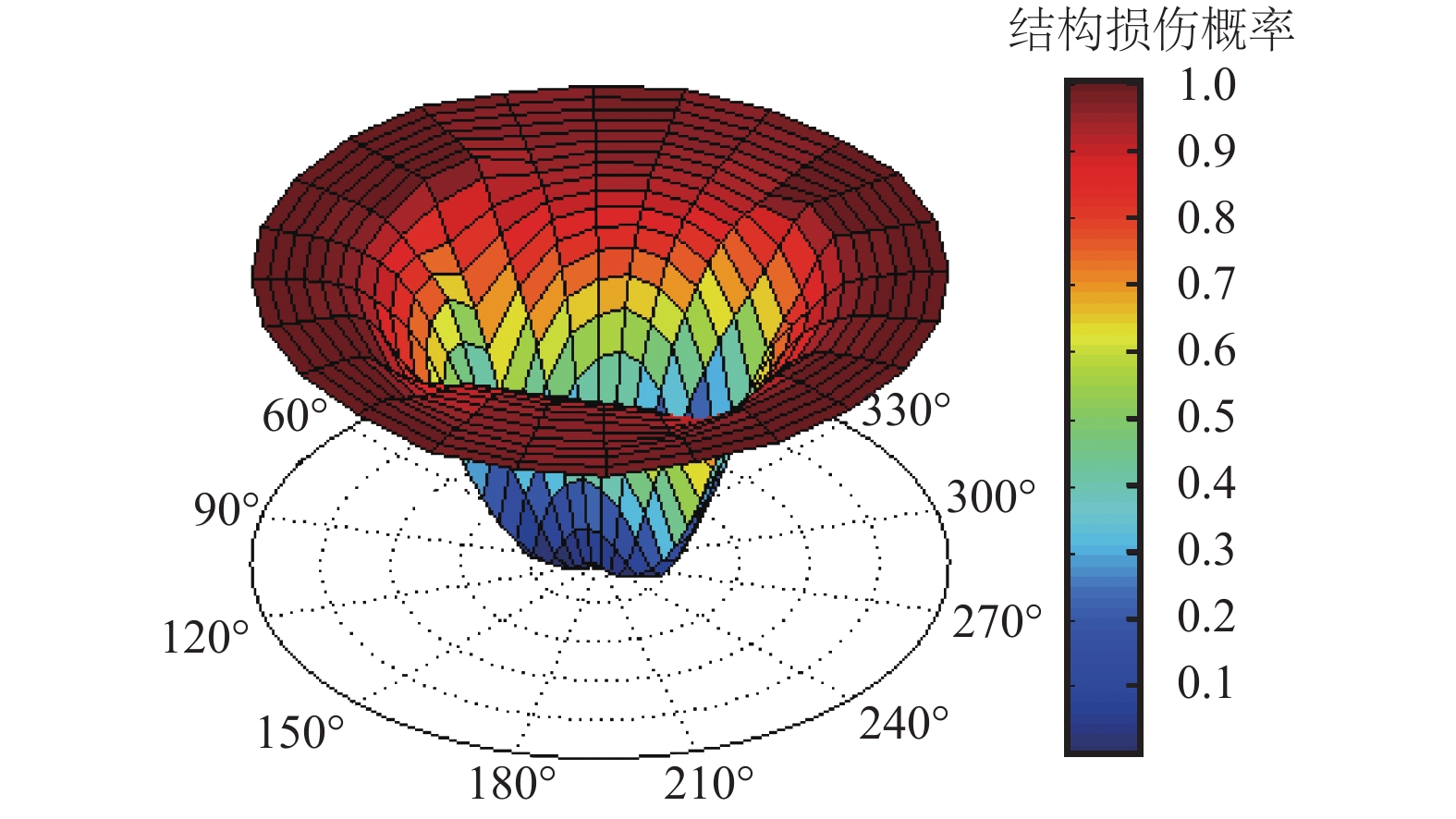
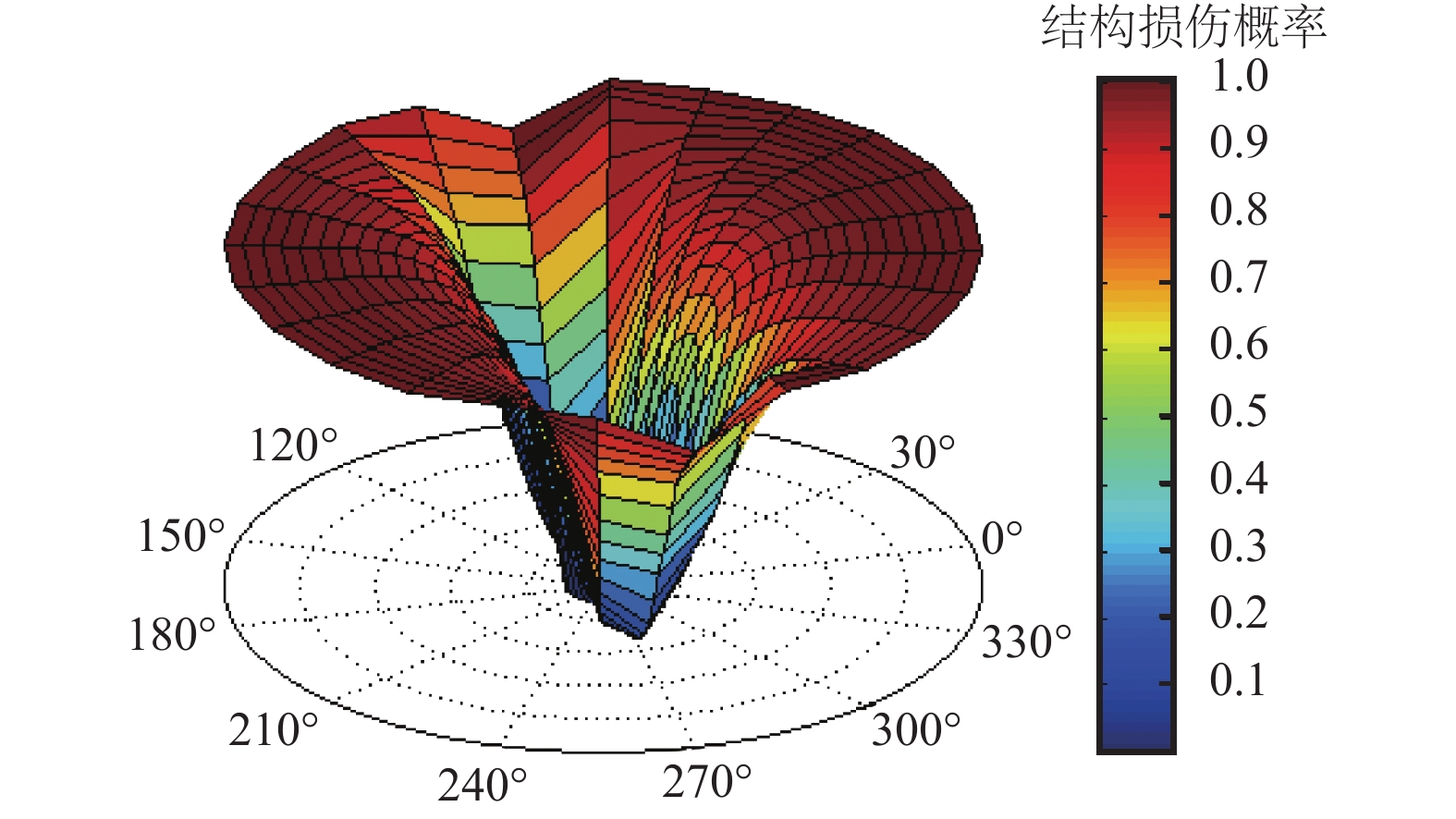
摘要: 为了评估桥梁结构近场抗震性能,建立了桥梁构件的三维地震易损性分析流程. 基于工程结构可靠度理论,用构件三维失效曲面表征墩柱、支座构件的损伤状态,将包含多个单一损伤指标的损伤状态方程作为三维地震易损性分析的损伤指标;其次在既有墩柱弯曲和剪切失效曲面研究的基础上,构建了墩柱弯曲和剪切破坏的损伤状态方程;并基于支座地震损伤的相对变形,建立了支座损伤状态的方程. 在此基础上,构建了墩柱和支座三维地震损伤状态的判别准则,并对不同损伤状态进行了量化. 结合各国桥梁抗震设计规范和工程结构可靠度理论,最后实现了三维地震易损性的计算分析. 通过一维地震易损性的简化验证,表明所提方法可用于桥梁结构的地震易损性分析中,并且所得结果与PSDA (probabilistic seismic demand analysis)法的最大概率偏差小于4%.Abstract: In order to evaluate the near-field seismic performance of bridge structures, a three-dimensional (3D) seismic vulnerability analysis process for bridge components is established. Based on the reliability theory of engineering structures, 3D failure surfaces of the components are used to characterize the damage status of pier columns and bearing components, and the damage state equation containing multiple independent damage indices is regarded as the damage index for the 3D seismic vulnerability analysis. In light of the existing research on the bending and shearing failure surfaces of piers, damage state equations for piers with bending failure and shear failure and for bearings with relative displacements are built. On this basis, the criteria for 3D seismic damage of pier columns and bearings are established, and quantitative methods for calculating the damage in different damage states are presented. The final calculation and analysis of the 3D seismic vulnerability of bridge components are accomplished in accordance with the bridge seismic design codes in various countries and the reliability theory of engineering structures. A simplified validation of one-dimensional seismic vulnerability shows that the proposed method can be applied to the seismic vulnerability analysis of bridge structures, with a maximum probability deviation of less than 4% from the traditional probabilistic seismic demand analysis (PSDA) method.
-
表 1 墩柱剪切破坏的损伤判别准则
Table 1. Criteria of pier shear damage
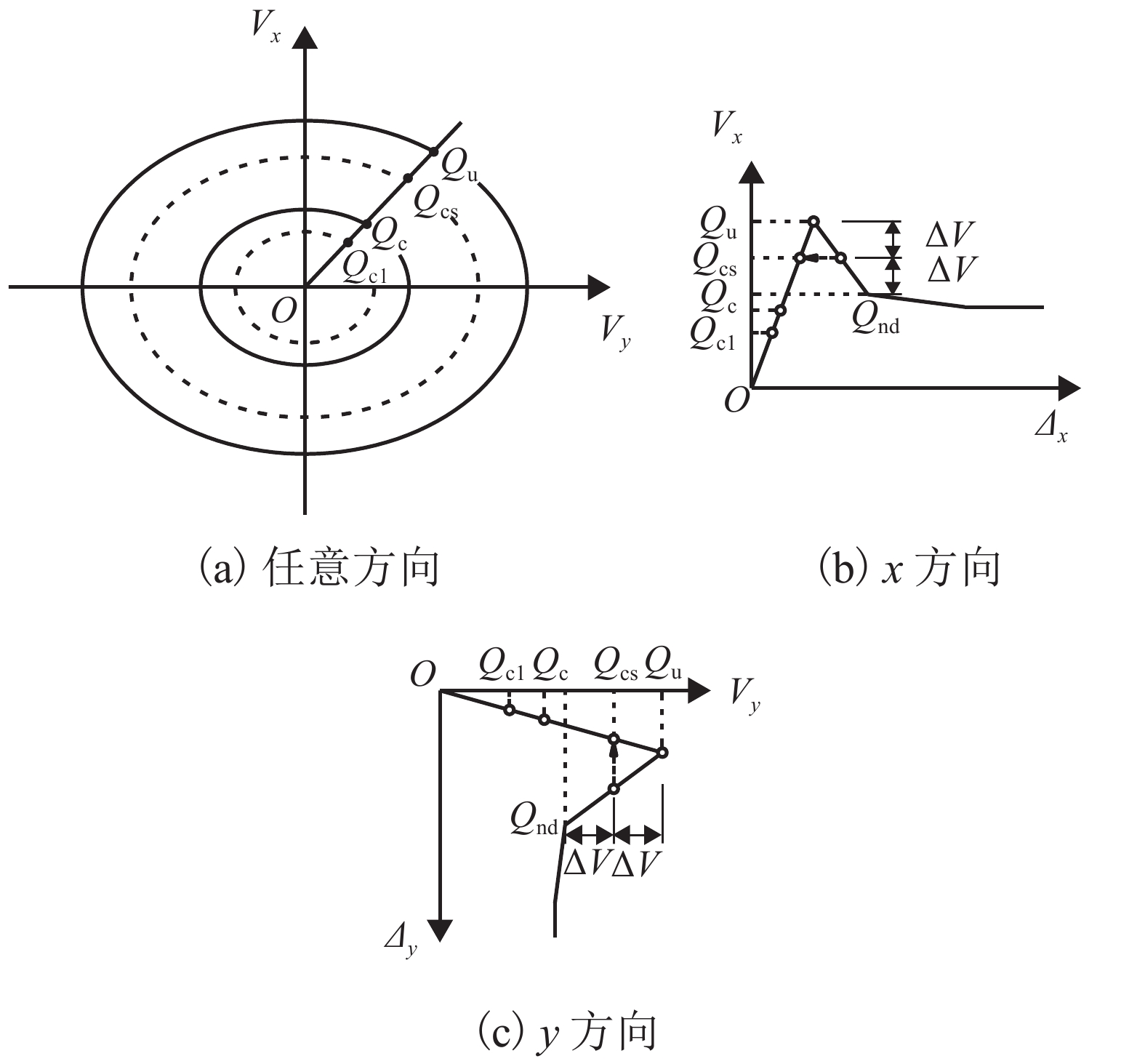
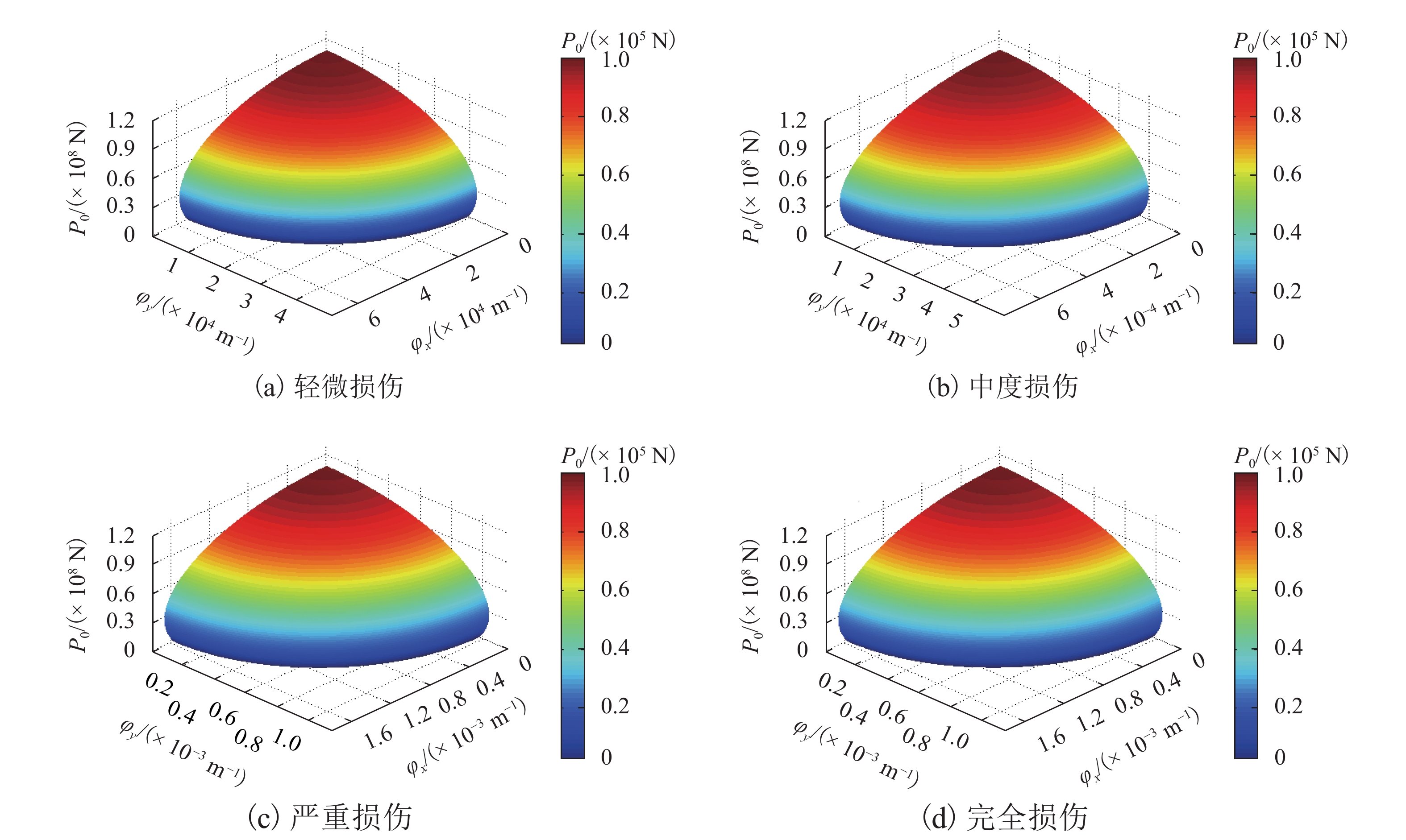
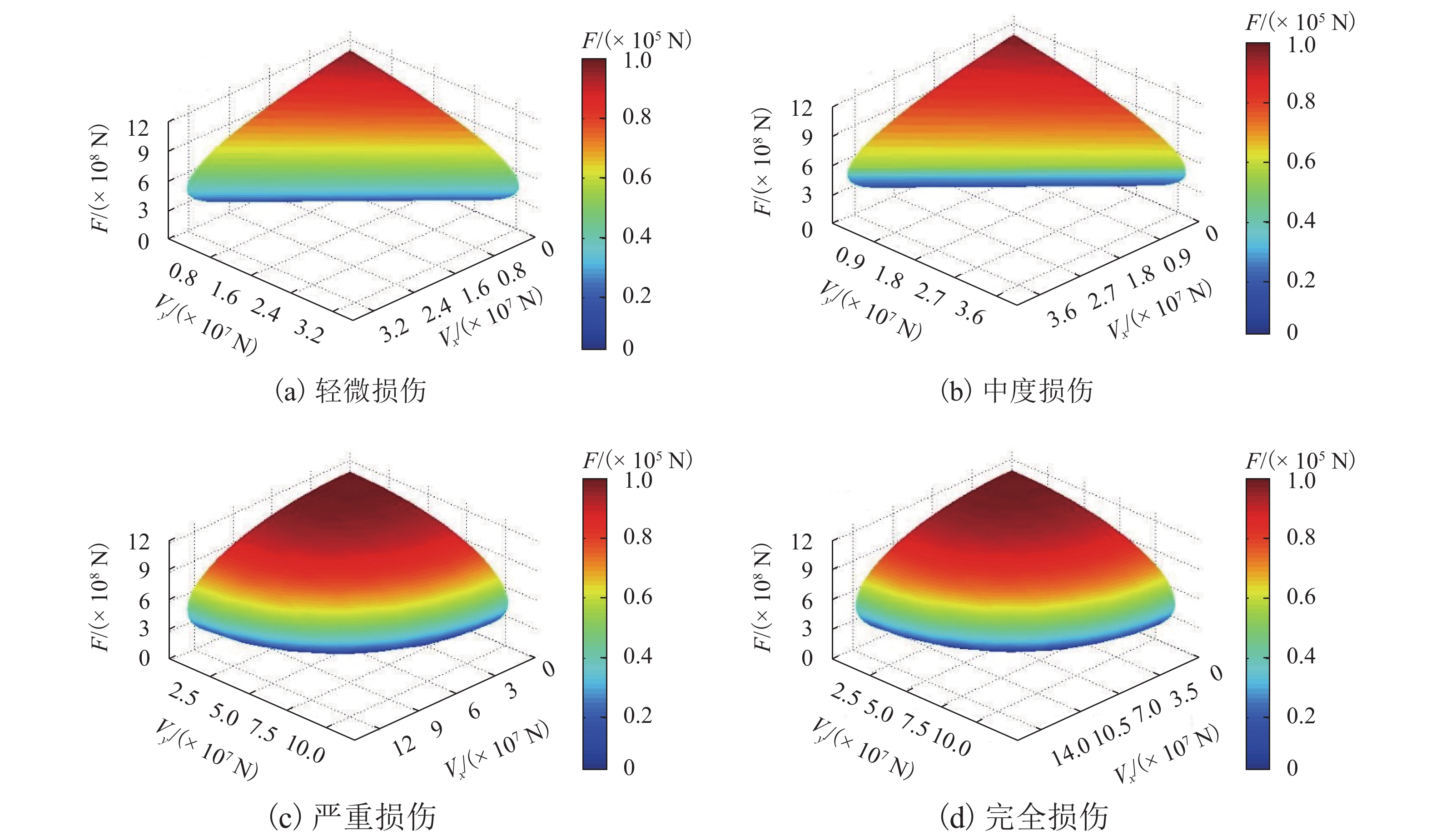
损伤状态 描述 指标 无损 仅出现微小裂缝 0 < V ≤ Qc1 轻微 微裂缝明显增多,
保护层轻微剥落Qc1 < V ≤Qc 中等 出现首条完整的斜裂缝(临界斜
裂缝),保护层剥落Qc < V ≤Qcs 严重 裂缝数量明显增加,出现较宽的
完整斜裂缝,保护层大面积剥落Qcs < V ≤ Qu 完全 箍筋断裂,核心混
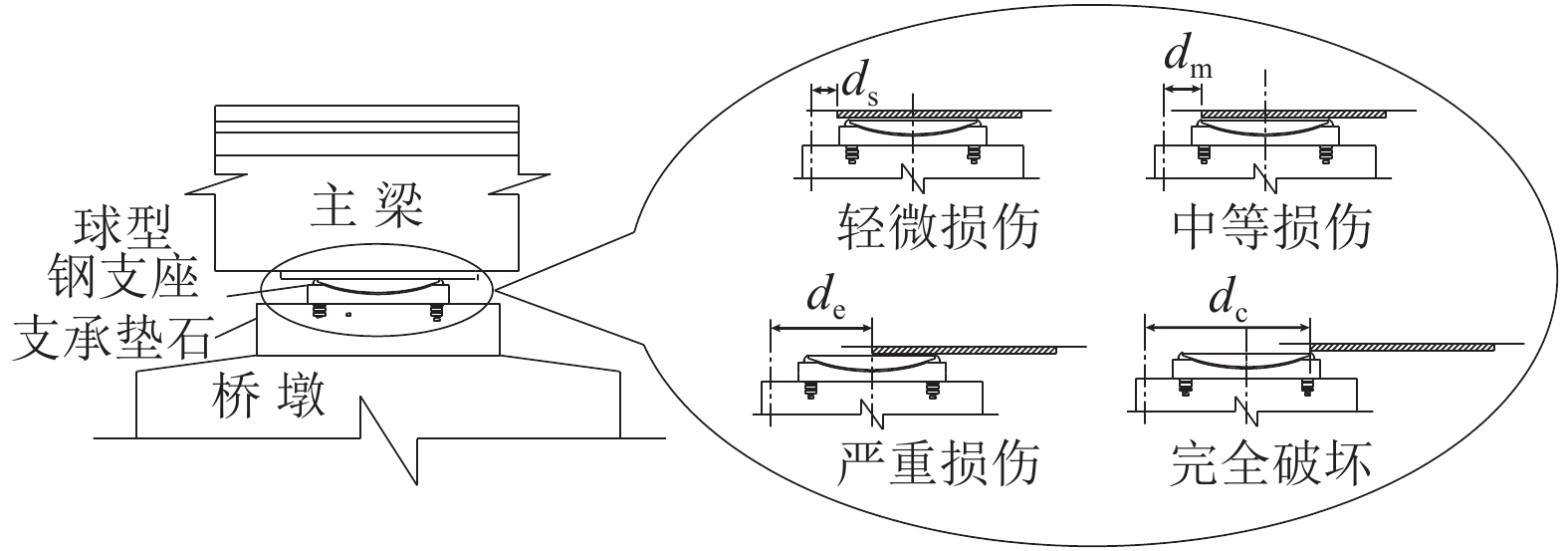
凝土压碎Qu < Q 表 2 支座顺桥向的地震损伤判别准则
Table 2. Longitudinal seismic damage criterion for bearings
损伤
状态描述 指标 无损 远离,上支座板位移小于设计位移值 0 < d < ds 轻微 远离,上支座板位移小于其边缘
到衬板边缘间的距离ds < d≤dm 中等 远离,上支座板位移小于其边缘
到衬板中心间的距离dm < d ≤ de 严重 远离,上支座板位移小于其边缘到衬板另一侧边缘间的距离 de < d ≤ dc 完全 远离,上支座板已从衬板滑落 d > dc 表 3 结构参数分布类型及分布特征值
Table 3. Distribution types of structural parameters and their characteristic values
结构参数 分布类型 分布参数 P1 P2 混凝土极限应变 对数正态 0.004 0.001 C35混凝土抗压强度/MPa 正态 28 4.5 C40混凝土抗压强度/MPa 正态 32 4.27 混凝土强度缩放系数 均匀 1.08 1.32 钢筋屈服强度/MPa 对数正态 5.99 0.1 钢筋应变硬化系数 均匀 0.04 0.06 钢筋弹性模量/GPa 对数正态 200 10.35 二期恒载缩放系数 均匀 0.9 1.1 混凝土容重/(kg•m–3) 均匀 22 500 27 500 支座水平承载力缩放系数 均匀 0.8 1.2 表 4 支座损伤指标
Table 4. Damage index values of bearings
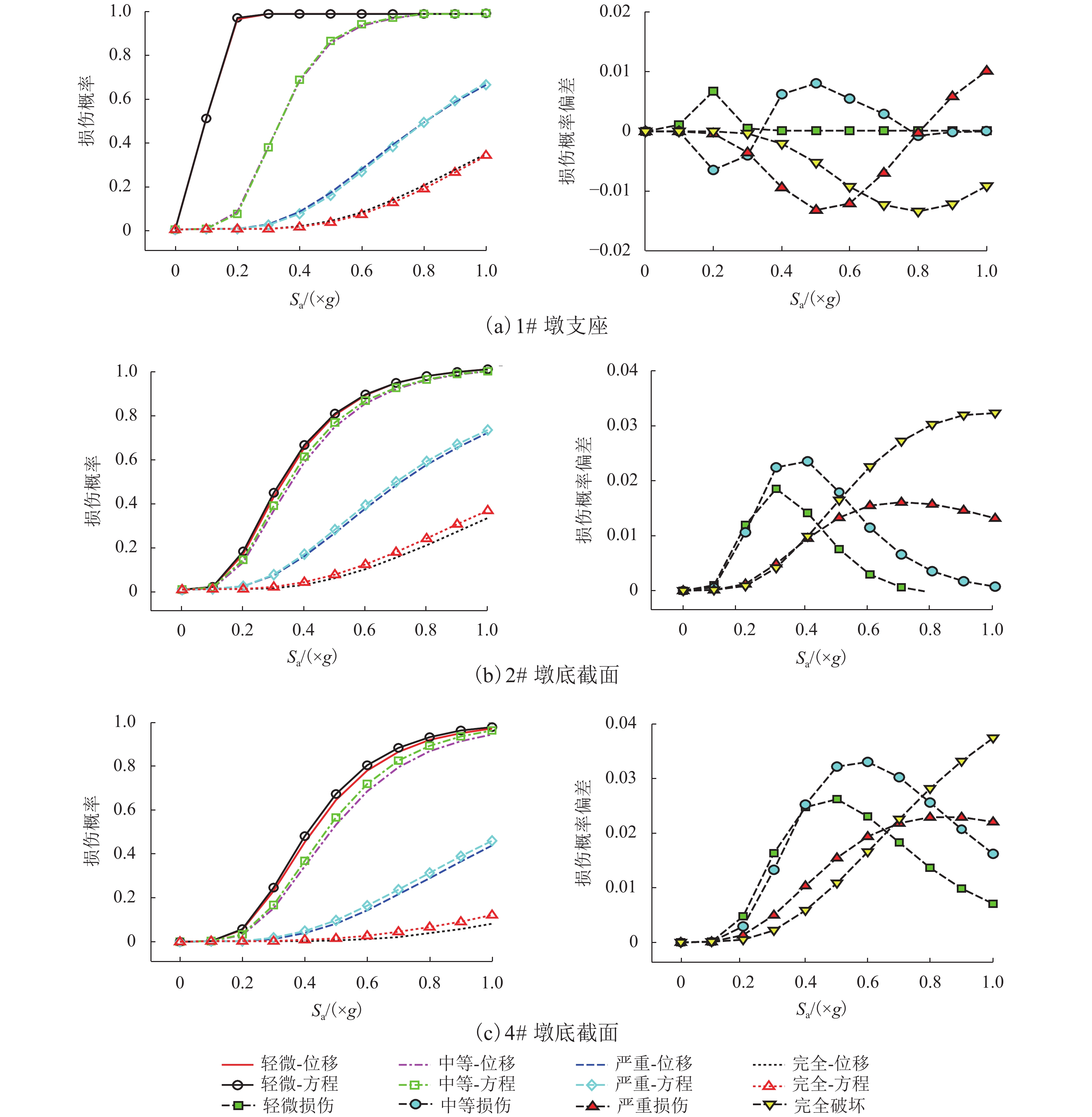
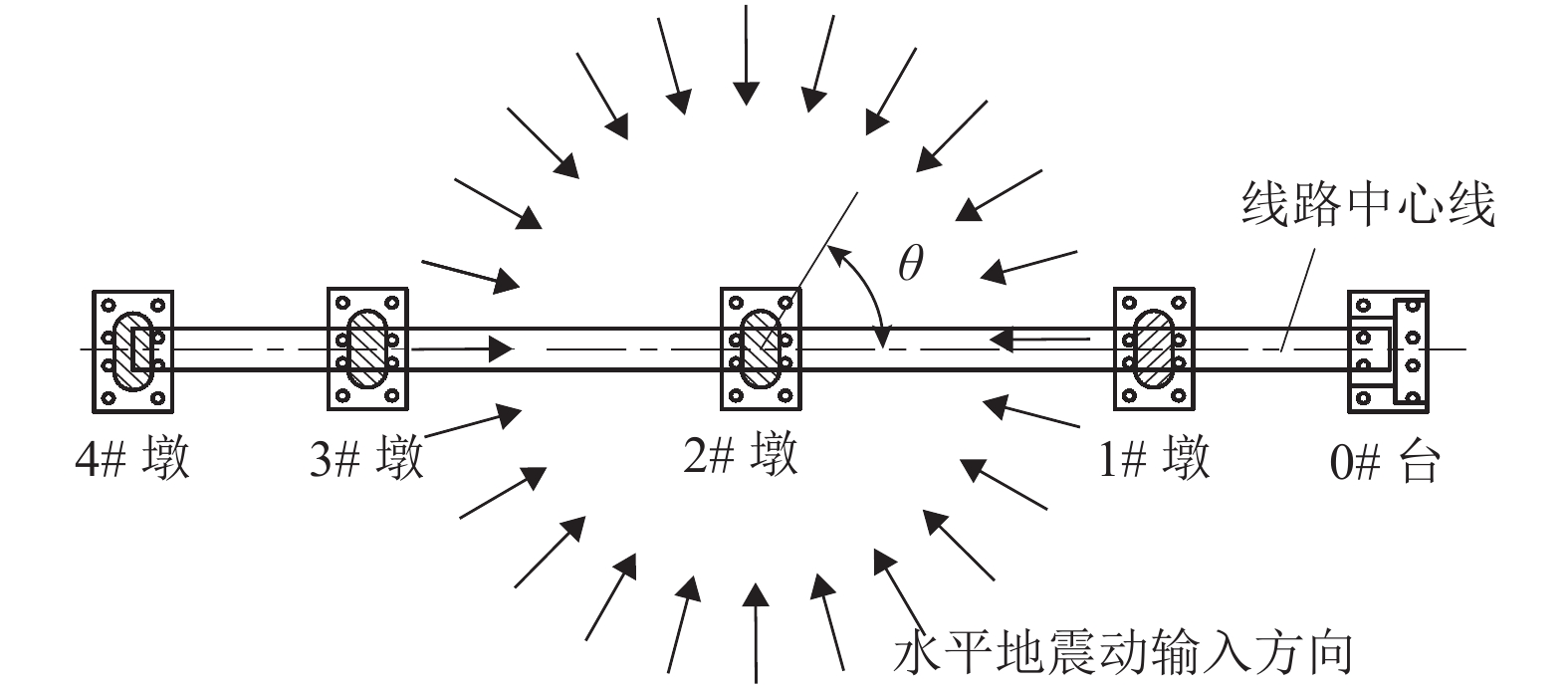
类别 损伤状态 0# 台支座 1# 墩支座 4# 墩支座 纵向 无损 D ≤ 150 D ≤ 100 D ≤ 100 轻微 150 < D ≤ 325 100 < D ≤ 430 100 < D ≤ 275 中等 325 < D ≤ 635 430 < D ≤ 1 225 275 < D ≤ 585 严重 635 < D ≤ 945 1 225 < D ≤ 2 020 585 < D ≤ 895 完全 D > 945 D > 2 020 D > 895 横向 无损 D ≤ 20 D ≤ 20 D ≤ 20 轻微 20 < D ≤ 135 20 < D ≤ 280 20 < D ≤ 135 中等 135 < D ≤ 445 280 < D ≤ 1 075 135 < D ≤ 445 严重 445 < D ≤ 755 1 075 < D ≤ 1 870 445 < D ≤ 755 完全 D > 755 D > 1 870 D > 755 表 5 构件概率地震需求模型参数表
Table 5. Parameters of probabilistic seismic demand model for components
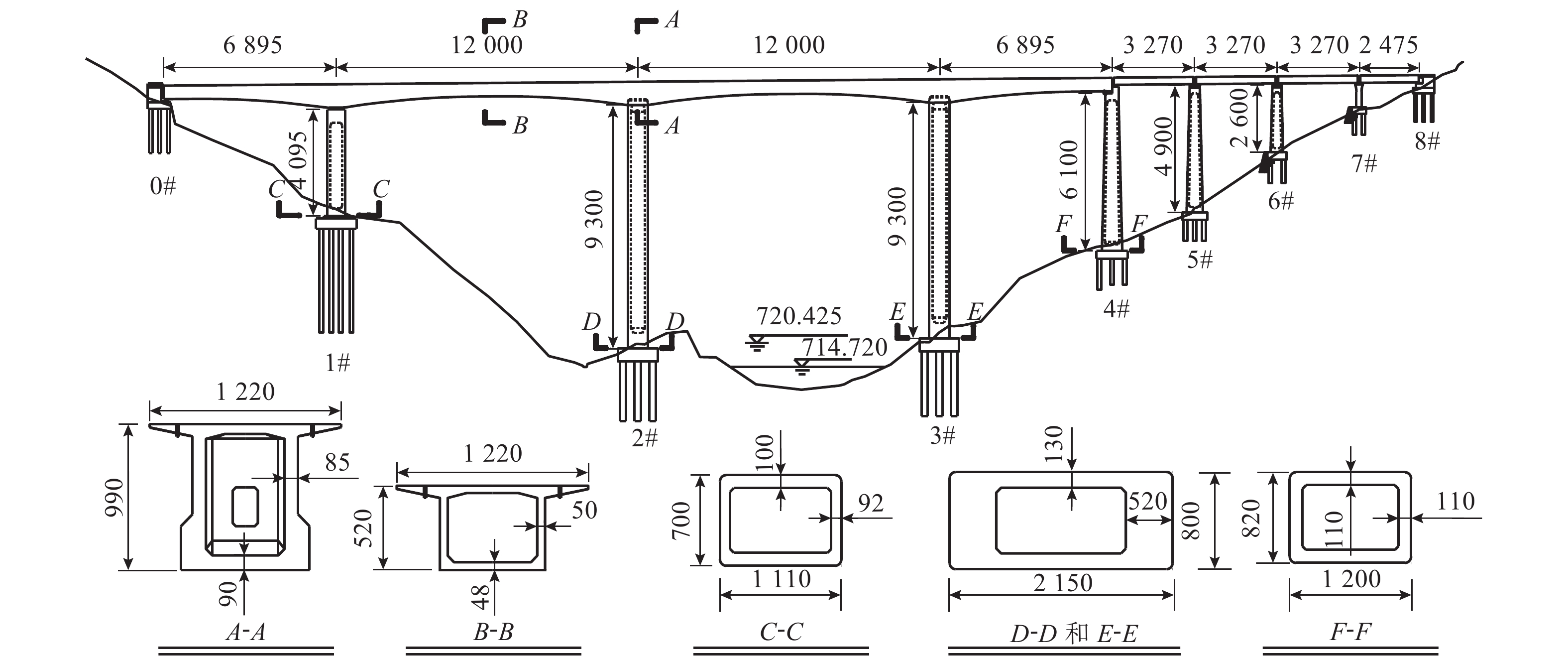
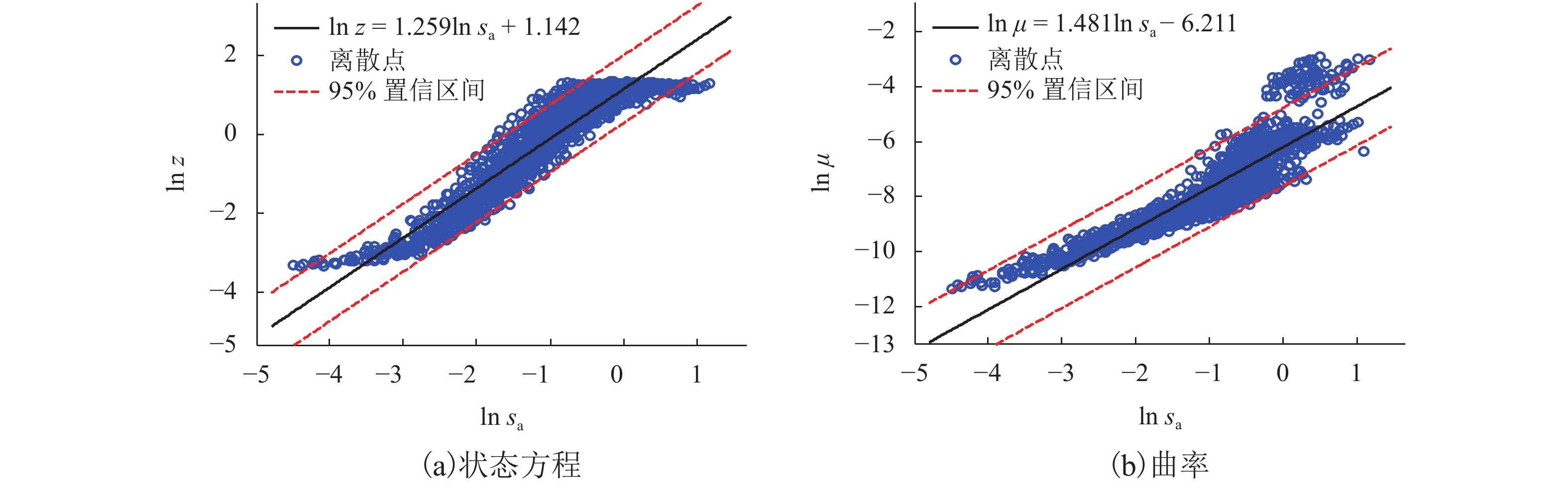
构件名称 损伤指标 标准差 拟合优度 R2 2# 墩底截面 φx 0.725 0.811 Z 0.437 0.895 4# 墩底截面 φx 0.516 0.857 Z 0.445 0.867 1# 墩支座 d 0.361 0.918 Z 0.421 0.923 -
单德山,张二华,董俊,等. 汶川地震动衰减特性及其大跨高墩连续刚构桥的地震响应规律[J]. 土木工程学报,2017,50(4): 107-115.SHAN Deshan, Zhang Erhua, Dong Jun, et al. Ground motion attenuation characteristics of Wenchuan earthquake and seismic response law of long-span continuous rigid frame bridge with high-rise pier[J]. China Civil Engineering Journal, 2017, 50(4): 107-115. THEODOULIDIS N, KARAKOSTAS C, LEKIDIS V, et al. The Cephalonia,Greece,January 26(M6.1)and February 3,2014(M6.0)earthquakes:near-fault ground motion and effects on soil and structures[J]. Bulletin of Earthquake Engineering, 2016, 14(1): 1-38. doi: 10.1007/s10518-015-9807-1 BILLAH A H M, ALAM M S. Seismic fragility assessment of highway bridges:a state-of-the-art review[J]. Structure and Infrastructure Engineering, 2015, 11(6): 804-832. doi: 10.1080/15732479.2014.912243 GIDARIS I, PADGETT J E, BARBOSA A R, et al. Multiple-hazard fragility and restoration models of highway bridges for regional risk and resilience assessment in the united states:state-of-the-art review[J]. Journal of Structural Engineering, 2017, 143(3): 04016188. doi: 10.1061/(ASCE)ST.1943-541X.0001672 STEFANIDOU S P, KAPPOS A J. Methodology for the development of bridge-specific fragility curves[J]. Earthquake Engineering & Structural Dynamics, 2017, 46(1): 73-93. 董俊,单德山,张二华,等. 非规则桥梁近、远场地震易损性对比分析[J]. 哈尔滨工业大学学报,2016,48(3): 159-165. doi: 10.11918/j.issn.0367-6234.2016.03.027DONG Jun, SHAN Deshan, ZHANG Erhua, et al. Near and far-field seismic fragility comparative analysis of irregular bridge[J]. Journal of Harbin Institute of Technology, 2016, 48(3): 159-165. doi: 10.11918/j.issn.0367-6234.2016.03.027 BILLAH A H M M, ALAM M S, BHUIYAN M A R. Fragility analysis of retrofitted multicolumn bridge bent subjected to near-fault and far-field ground motion[J]. Journal of Bridge Engineering, 2013, 18(10): 992-1004. doi: 10.1061/(ASCE)BE.1943-5592.0000452 LIN C J, HUNG H, LIU K, et al. Reconnaissance observation on bridge damage caused by the 2008 Wenchuan (China) earthquake[J]. Earthquake Spectra, 2010, 26(4): 1057-1083. doi: 10.1193/1.3479947 KAWASHIMA K, TAKAHASHI Y, GE H, et al. Damage of bridges in 2008 Wenchuan,China,earthquake[J]. Journal of Earthquake Engineering, 2009, 13: 956-998. LI Jianzhong, PENG Tianbo, XU Yan. Damage investigation of girder bridges under the Wenchuan earthquake and corresponding seismic design recommendations[J]. Earthquake Engineering and Engineering Vibration, 2008, 7(4): 337-344. doi: 10.1007/s11803-008-1005-6 KHALED M. Safety and reliability of bridge structures[M]. New York : CRC Press, 2009: 335-348 中华人民共和国交通运输部. 公路桥梁抗震设计细则: JTG/T B02-01—2008[S]. 北京: 人民交通出版社, 2008 California Department of Transportation (Caltrans). Seismic design criteria: version1.6[S]. California: [s.n.], 2010 BRESLER B. Design criteria for reinforced concrete columns under axial load and biaxial bending[J]. ACI Journal, 1960, 57(11): 481-490. VECCHIO F, COLLINS M. The modified compression-field theory for reinforced concrete eleImproving the performance of sodium silicate for track slab by promoting polymerization and biomineralizationments subjected to shear[J]. ACI Structural Journal, 1986, 83(1): 19-31. JANGID R S. Seismic reponse of sliding structures to bidirectional earthquake excitation[J]. Earthquake Engineering and Structural Dynamics, 1996, 25(11): 1301-1305. doi: 10.1002/(ISSN)1096-9845 PARK H G, YU E J, CHOI K. Shear strength degradation model for rc columns subjected to cyclic loading[J]. Engineering Structures, 2012, 34(1): 187-197. PARK R, PAULAY T. Reinforced concrete structures[M]. New York: John Wiley & Sons, 1975: 118-194 赵国藩. 高等钢筋混凝土结构学[M]. 北京: 机械工业出版社, 2005: 16-127 PRIESTLEY M J N, VERMA R, XIAO Y. Seismic shear strength of reinforced concrete columns[J]. ASCE Journal of Structural Engineering, 1994, 120(8): 2310-2329. PRIESTLEY M J N, SEIBLE F, CALVI G M. Seismic design and retrofit of bridges[M]. New York: John Wiley & Sons, 1996: 280-347 RICLES J M.YANG Y S, PRIESTLEY M J N. Modeling non-ductile R/C columns for seismic analysis of bridges[J]. Journal of Structural Engineering, 1998, 124(4): 415-425. doi: 10.1061/(ASCE)0733-9445(1998)124:4(415) GALAL K E, GHOBARAH A. Flexural and shear hysteretic behaviour of reinforced concrete columns with variable axial load[J]. Engineering Structures, 2003, 25(1): 1353-1367. 中华人民共和国铁道部. 铁路工程抗震设计规范: GB 50111—2006[S]. 北京: 中国计划出版社, 2006 铁道部第三勘测设计院. 铁路桥涵地基和基础设计规范: TB10002.5—2005[S]. 北京: 中国铁道出版社, 2005 BAKER J W. Quantitative classification of near-fault ground motions using wavelet analysis[J]. Bulletin of the Seismological Society of America, 2007, 99(5): 1486-1501. MEHDIZADEH M. Uncertainty treatment in performance based seismic assessment of typical bridge classes in united states[D]. Orlando: University of Central Florida, 2014 ALAM M S, BHUIYAN M A R, BILLAH A H M M. Seismic fragility assessment of sma-bar restrained multi-span continuous highway bridge isolated by different laminated rubber bearings in medium to strong seismic risk zones[J]. Bulletin of Earthquake Engineering, 2012, 10(6): 1885-1909. doi: 10.1007/s10518-012-9381-8 期刊类型引用(4)
1. 王东升,童磊,王荣霞,孙治国. 大跨PC连续刚构桥抗震研究进展综述. 西南交通大学学报. 2023(03): 511-526 .  本站查看
本站查看2. 李佳璐,任乐平,胡伟. Nataf变换的桥梁系统多维地震易损性分析方法. 交通运输工程学报. 2022(01): 82-92 .  百度学术
百度学术3. 单德山,苑洁艺,董俊,李乔. 桥梁构件三维地震易损性分析. 铁道学报. 2021(09): 133-142 .  百度学术
百度学术4. 单德山,韩璐璐,瞿发宪,董俊. 地震动入射角对空心薄壁高墩桥梁地震易损性的影响. 交通运输工程学报. 2020(06): 90-103 .  百度学术
百度学术其他类型引用(19)
-





 下载:
下载:


 百度学术
百度学术
