磁悬浮铣削电主轴时变质量系统旋转惯性特性及动态响应研究

康辉民; 席家乐; 刘厚才; 段良辉

doi:10.3969/j.issn.0258-2724.20240295

磁悬浮铣削电主轴时变质量系统旋转惯性特性及动态响应研究

doi: 10.3969/j.issn.0258-2724.20240295

1.
湖南科技大学机电工程学院，湖南湘潭 411201
2.
江南工业集团有限公司数控加工分厂，湖南湘潭 411207

基金项目: 国家自然科学基金项目（51875198）；湖南省教育厅重点项目（23A0360）

详细信息

作者简介:
康辉民（1975—），男，教授，博士，研究方向为转子系统动力学控制，E-mail：xykanghm@163.com

通讯作者:
刘厚才（1975—），男，副教授，博士，研究方向为机械系统设计与结构优化，E-mail：houcailiu@163.com

中图分类号: TH113
计量
- 文章访问数: 58
- HTML全文浏览量: 27
- PDF下载量: 9
- 被引次数: 0
出版历程
- 收稿日期: 2024-06-19
- 修回日期: 2024-09-09
- 网络出版日期: 2025-03-06

Rotational Inertial Characteristics and Dynamic Response of Motorized Spindle Time-Varying Mass System for Magnetic Suspension Milling

1.
School of Mechanical Engineering, Hunan University of Science and Technology, Xiangtan 411201, China
2.
Numerical Control Processing Branch of Jiangnan Industrial Group Co., Ltd., Xiangtan 411207, China

摘要

摘要:
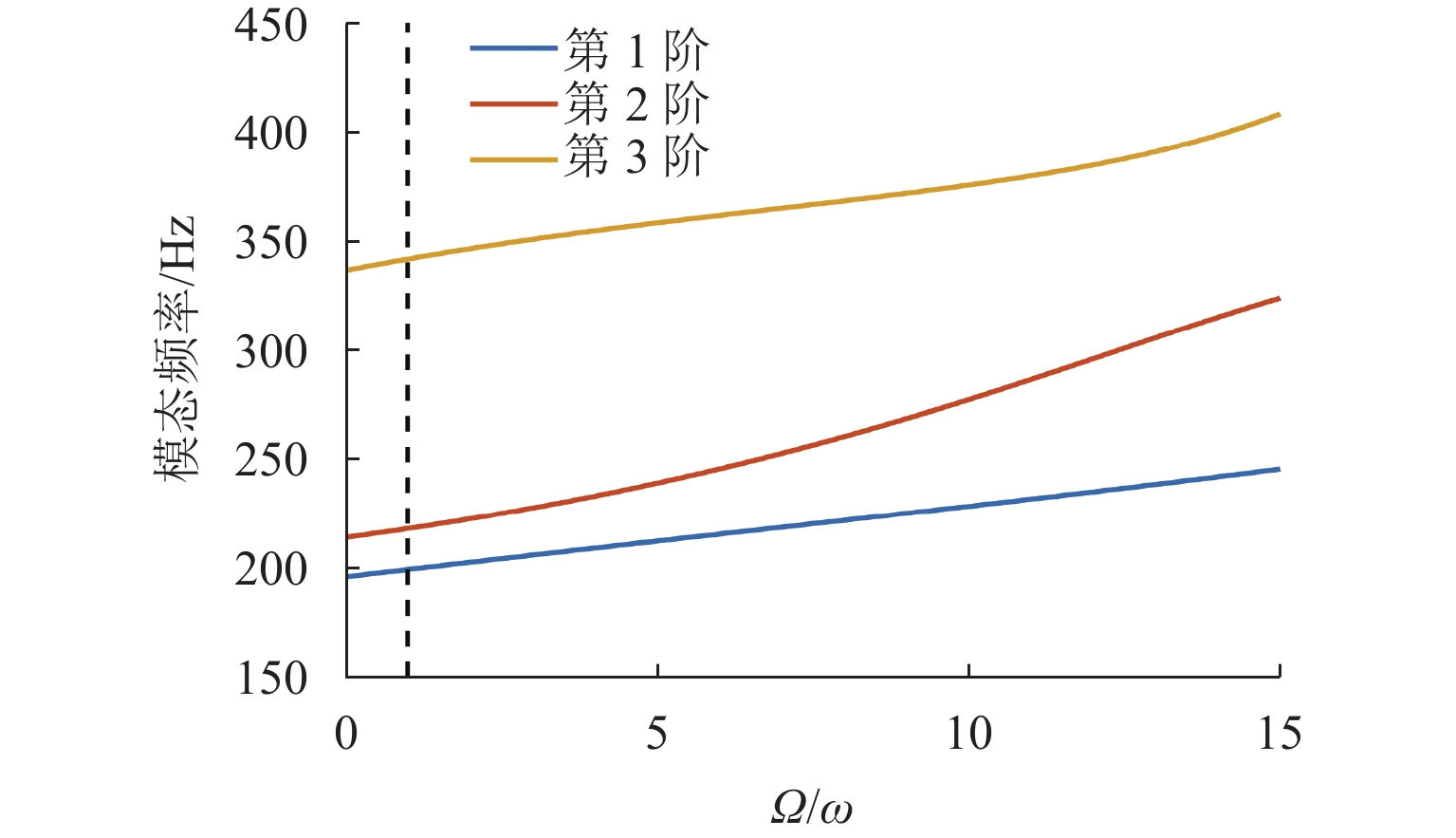
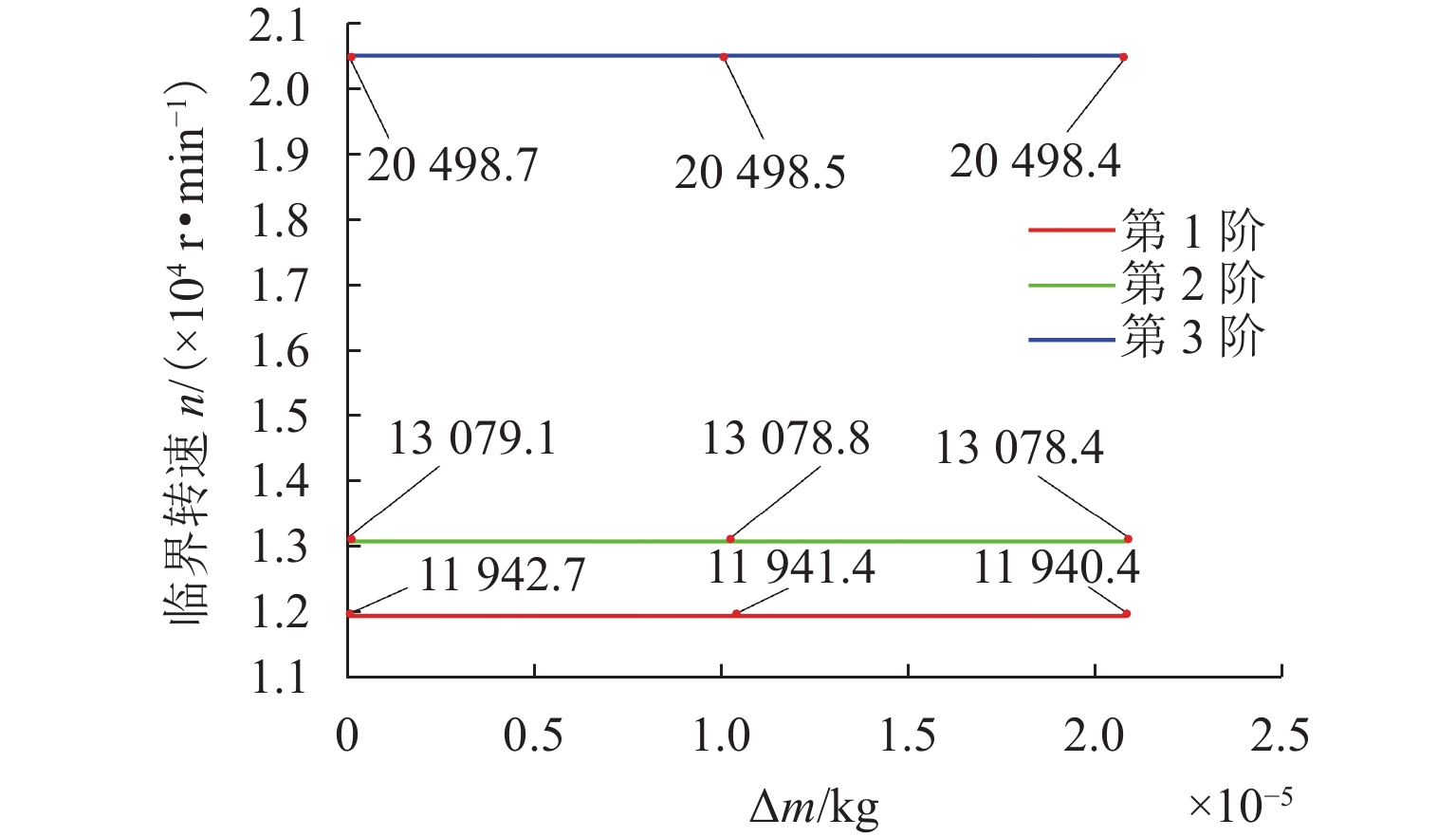
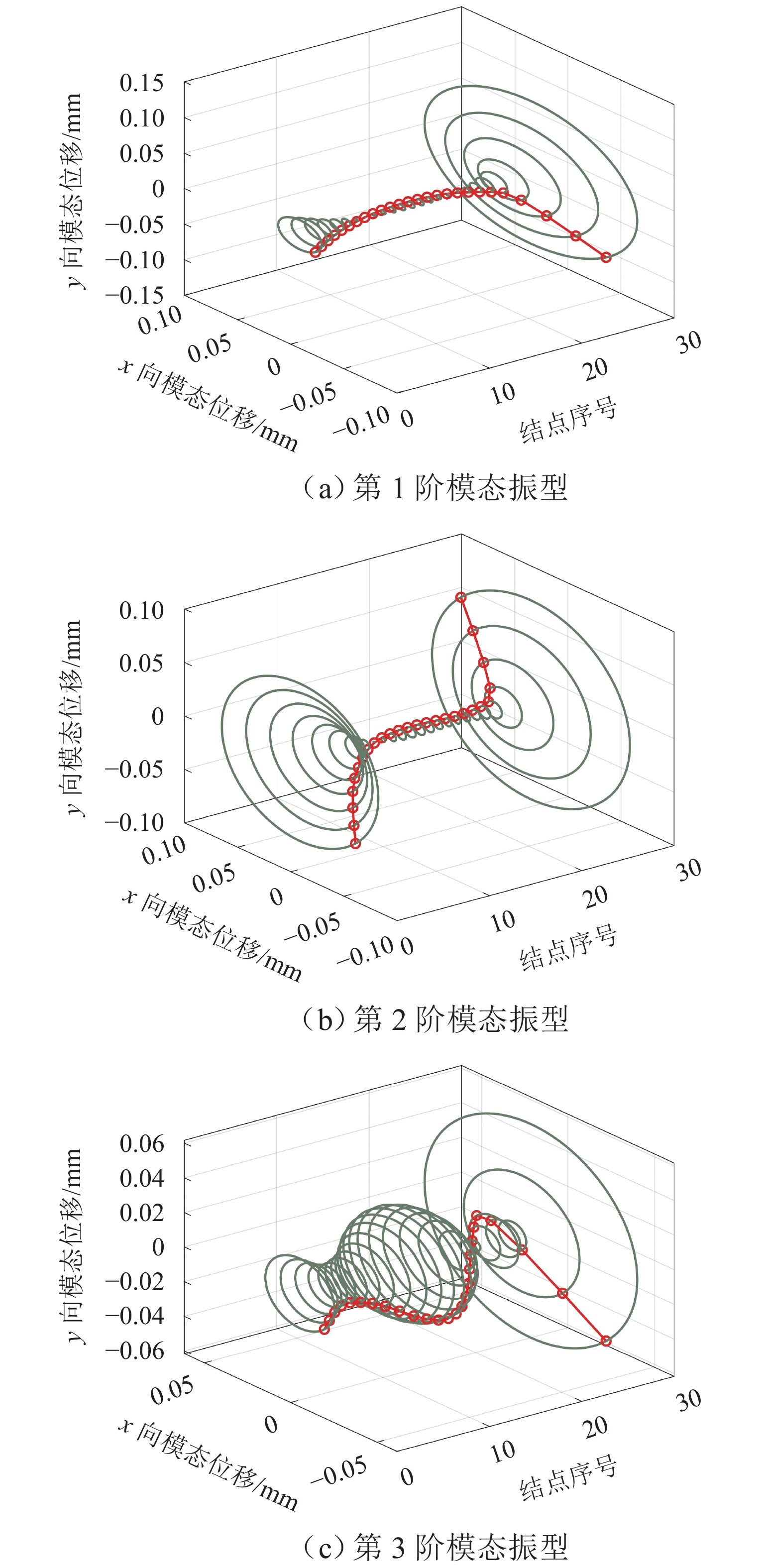
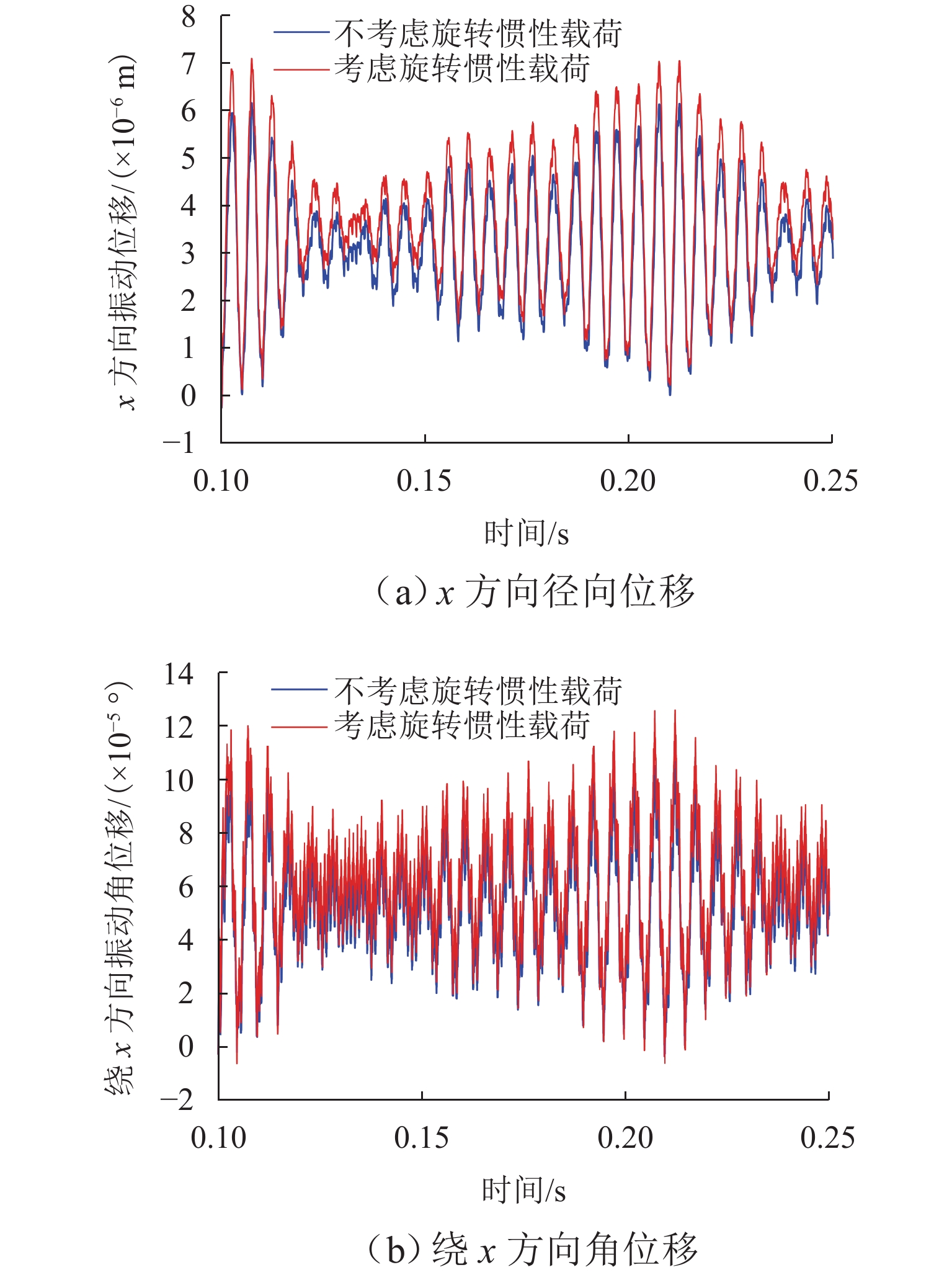
针对磁悬浮铣削电主轴在切削加工过程中因切屑不断进入、离开刀具容屑槽导致系统质量大小和分布不断变化，进而引起系统动力学特性的非线性变化问题. 首先，依据金属连续切削原理求得单个切屑质量，并结合连续梁振动理论，应用有限单元法建立“磁悬浮轴承-电主轴-刀具-切屑”时变质量系统的动力学模型；然后，采用龙格库塔法对系统的运动微分方程进行求解，分析切屑从进入到离开容屑槽的整个过程，切屑质量变化对系统固有频率、振型的影响规律；进而探索由时变切屑质量所引起的旋转惯性载荷、陀螺力矩、切削力、磁悬浮轴承电磁力等激励下的系统振动响应规律；最后，利用MATLAB软件对系统进行仿真求解. 结果表明：切屑质量从0增大到2.08 × 10⁻⁵ kg时，系统前三阶临界转速分别下降约2.3、0.7、0.3 r/min，可知时变切屑质量对系统固有特性影响较小；旋转惯性载荷对系统的动态响应有较大影响，尤其是对切削加工点，使切削点的径向振动响应和角向振动响应的幅值出现0～9.7 × 10⁻⁷ m和0～2.5 × 10⁻⁵ rad不等的增大，还使加工点处径向振动和角向振动平衡位置的偏移距离分别增加约5.1 × 10⁻⁷ m和9.3 × 10⁻⁶ rad.
- 磁悬浮电主轴 /
- 时变质量 /
- 旋转惯性特性 /
- 固有特性分析 /
- 振动响应
Abstract:
As chips continuously enter and leave from the tool flute during the cutting process of the motorized spindle for magnetic suspension milling, the size and distribution of the system mass are constantly changing, leading to the nonlinear change of system dynamics characteristics. To address these issues, firstly, the mass of a single chip was calculated based on the principle of continuous metal cutting, and then combined with the theory of continuous beam vibration, the dynamics model of the “magnetic suspension bearing–motorized spindle–tool–chip” time-varying mass system was established by using the finite element method. Secondly, the Runge-Kutta method was used to solve the differential equation of motion of the system, and the influence of chip mass change on the natural frequency and mode shape of the system was analyzed during the entire process covering the chip’s entry into and leave from the tool flute. Then, the vibration response patterns of the system under the excitation of rotational inertial load, gyroscope torque, cutting force, and electromagnetic force of magnetic suspension bearings caused by time-varying chip mass were explored. Finally, the MATLAB software was used to simulate and solve the system. The results show that as the chip mass increases from 0 to 2.08 × 10⁻⁵ kg, the system’s first three critical speeds decrease by about 2.3, 0.7, and 0.3 r/min, respectively, indicating that the time-varying chip mass has a small effect on the system’s inherent characteristics. The rotational inertia load has a significant impact on the system’s dynamic response, especially at the cutting point, causing the radial vibration response and angular vibration response amplitudes at the cutting point to increase by 0–9.7 × 10⁻⁷ m and 0–2.5 × 10⁻⁵ rad, respectively, and it makes the radial vibration and angular vibration equilibrium positions at the cutting point to increase by about 5.1 × 10⁻⁷ m and 9.3 × 10⁻⁶ rad, respectively.
- motorized spindle of magnetic suspension /
- time-varying mass /
- rotational inertial characteristic /
- inherent characteristic analysis /
- vibration response

HTML全文

柴油机有热效率高、功率范围宽和可靠性高等优点，作为动力源广泛应用于各类交通工具. 然而，随着环境污染和化石资源的不断消耗，世界各国对柴油机的经济性和排放性提出了越来越高的要求^[1]. 从喷孔喷出油束的喷油特性直接影响柴油和空气的混合特性，特别是喷孔的喷油稳定性会直接影响每次喷射的循环喷油量以及油束喷雾在缸内的分布，从而对柴油机缸内燃烧过程产生重要的影响^[2]. 因此，喷油器的喷油规律以及喷油的稳定性对柴油机进行缸内喷雾、燃烧性能优化具有重要的意义^[3].

学者们针对喷油器喷孔喷油速率开展了大量研究工作，取得了丰富的研究成果^[4-6]. Suh等^[7]采用Bosch喷油规律测量方法，开展了电磁驱动式和压电驱动式喷油器喷油规律实验研究，实验发现，在同一工况条件下，压电式喷油器的循环喷油量高于电磁式喷油器. Payri等^[8]基于Bosch法开展了柴油和汽油为燃料的喷油速率实验研究发现，在相同的喷射条件下，柴油的喷射速率高于汽油的，这是由于柴油和汽油密度不同造成的. Postrioti等^[9]采用Zeuch和Bosch 2种方法进行喷油规律测量结果准确性的比较，结果表明，Bosch法测量的喷油开始时刻比Zeuch法测量的喷油始点延迟50～70 μs. Cavicchi等^[10]采用Zeuch法结合激光多普勒测振仪开展了喷油速率与针阀升程的实验研究发现，在喷油器电流信号加载后第70 μs时才测量到针阀的位移，且在针阀抬起的瞬间喷油速率出现一个下降的趋势，这是因为在针阀抬起后，喷孔内的燃油出现了短暂的回流，在针阀开启8～10 μs后喷油速率才得以上升. Desantes等^[11]基于动量法开展了生物质-柴油的混合油喷油规律实验，研究发现，在不同的工况下，喷油器喷孔的最大喷油速率随生物质掺混比的上升而增大. Luo等^[12]基于动量法对多喷孔喷油器的喷油规律进行了同步测量，对于直径相同的喷孔，喷孔的水平夹角越小，喷油起始时间会越晚，且喷油量会越小. Faghani等^[13]采用动量法对天然气直喷喷射器的稳定性进行研究，随着喷射脉宽的增加，相同工况下喷射的稳定性会上升，喷气规律曲线间的差异性会降低.

和传统的喷油规律测量方法相比，动量法是唯一可以测量多喷孔喷油器各孔喷油速率的实验方法，但较少有研究涉及到利用动量法对多孔喷油器在不同喷油压力下的稳定性. 因此，本文首先根据动量法开发了多孔喷油器各孔喷油规律测量装置；然后，基于研发的测量装置，在不同的负荷工况下，测量喷油器各喷孔喷油量的差异；在不同的喷油压力下，针对单一喷孔进行了喷孔喷油特性的研究，揭示了喷油压力对喷油量稳定性的影响机制.

1. 动量法测量原理和喷油稳定性评价

1.1 基于动量法的喷油规律测量原理

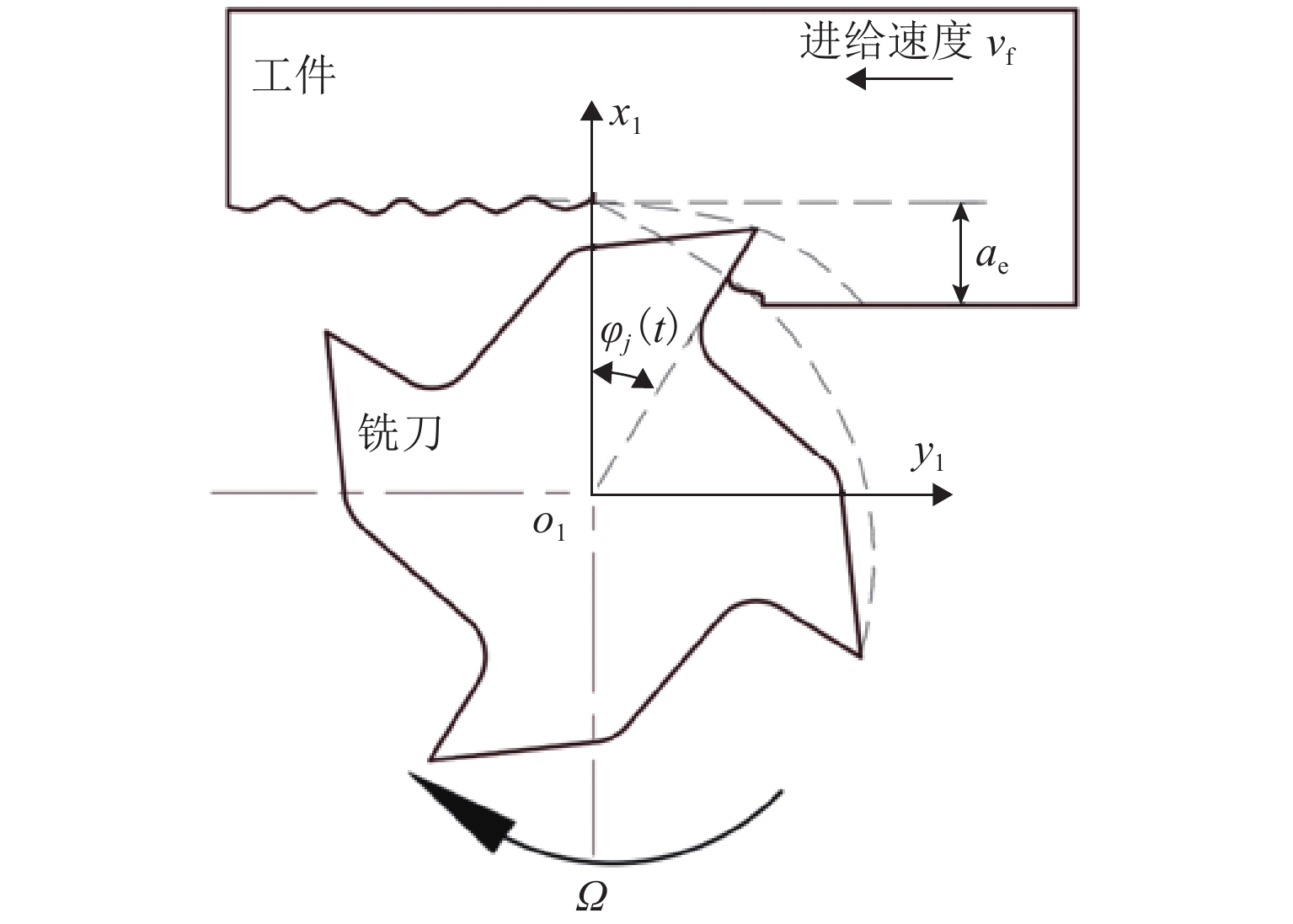
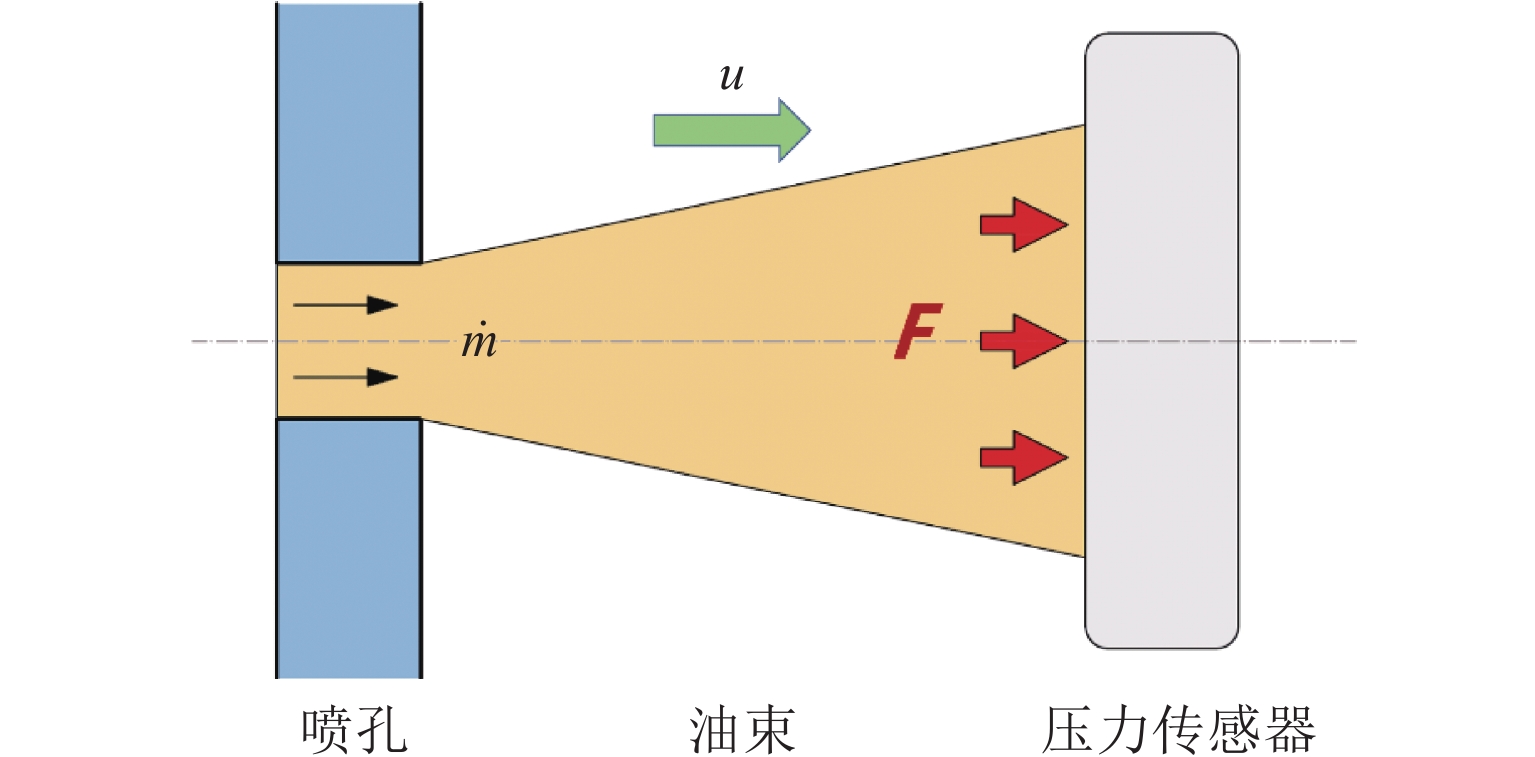
图1所示为动量法测量喷油规律原理示意. 图中：u为燃油的流速，$ \dot m $为喷孔内燃油瞬态质量， F为冲击力. 采用动量法时，在喷孔出口处一定距离上布置力传感器，力传感器表面与喷孔表面平行，此时假设油束完全撞击到传感器表面.

图 1 动量法测量喷油规律示意

Figure 1. Injection pattern measured by momentum method

下载: 全尺寸图片幻灯片

根据质量守恒定理，喷孔内燃油瞬态质量流量为

$\dot m = \rho uA ,$

(1)

式中：ρ为燃油密度，A为喷孔的流通面积.

通过传感器采集到瞬态冲击力，根据动量守恒定理可知，冲击力F为

$F=\dot{m}u.$

(2)

联立式（1）、（2），得到

$\dot m = \sqrt {\rho A F} .$

(3)

1.2 喷油量稳定性评价指标

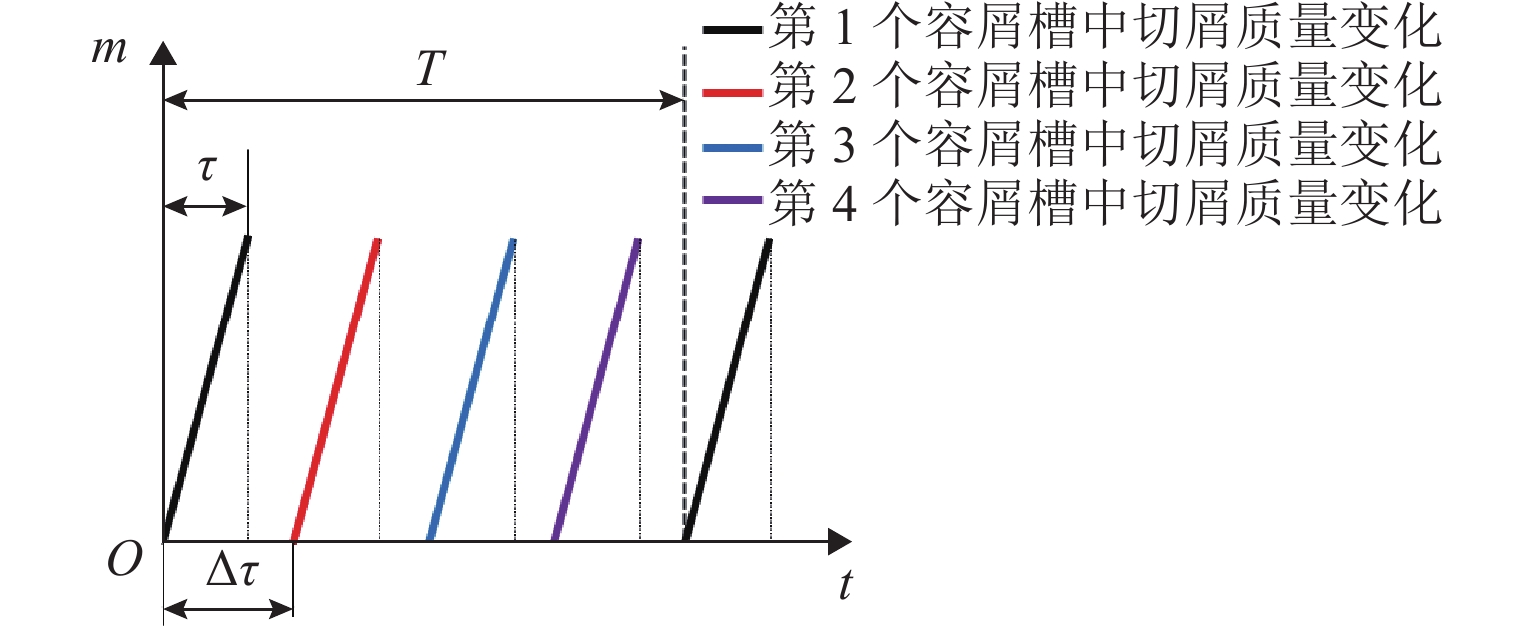
对于共轨多孔喷油器而言，喷孔与喷孔之间由于加工误差和流动的不一致性会导致喷油器各喷孔间燃油喷油量的差异. 此外，对于同一个喷孔，不同循环次数下，每次喷射的喷油量也存在不一致性现象. 因此，本文定义喷油量波动率σ来评价喷孔喷油量的稳定性，如式（4）.

$\sigma = \frac{{|{m_{{i}}} - {m_{{\text{ave}}}}|}}{{{m_{{\text{ave}}}}}} ,$

(4)

式中：m_i为第i个喷孔的喷油量，m_ave为总的喷油量的平均值.

2. 动量法测量系统设计

2.1 力传感器参数确定

据伯努利方程和动量定理，可计算出在最大压差下喷孔油束喷雾的理论最大冲击力为

${F_{{\text{max}}}} = \rho u_{\max }^2 A = 2 ({P_1} - {P_2}) A ,$

(5)

式中：P₁为喷油压力，P₂为喷孔出口背压，u_max为油束在喷孔处的流速.

在本文的实验条件下，P₁=120 MPa，P₂=0.1 MPa，计算得到F_max=17.03 N. 由于喷油持续时间为毫秒级，瞬态力传感器需要具有高的响应频率. 考虑到测量范围和响应频率要求，选择了大洋公司DYZ-100型力传感器作为本文实验用的瞬态力传感器，具体参数如表1所示.

表 1 力传感器主要性能指标

Table 1. Main performance indicators of force sensor

指标	数值
量程/N	0～20
输出精度/%	0.05
响应频率/kHz	10
工作温度/℃	−20～80

下载: 导出CSV

| 显示表格

2.2 喷油规律计算程序

在进行喷油规律计算时，首先通过数据实时采集子程序进行油束力信号采集，为保证对信号的完整采集，采样率设置为100 KS/s，同时根据2 Hz的喷油周期设置0.5 s的采样时间，并采用循环结构进行信号的连续采集；完成信号采集后，通过滤波子程序对采集到的数据进行滤波，以减少干扰杂波，在进行滤波时，根据FFT（Fast Fourier Transform）频谱分析结果发现，试验中主要的波形集中在0～1500 Hz频率段，因此，选择1500 Hz低通滤波以抑制高频噪声；最后，根据式（3）编程得到瞬态质量流量，在喷油持续期内进行积分处理，得到循环喷油量.

3. 装置测量结果准确性验证

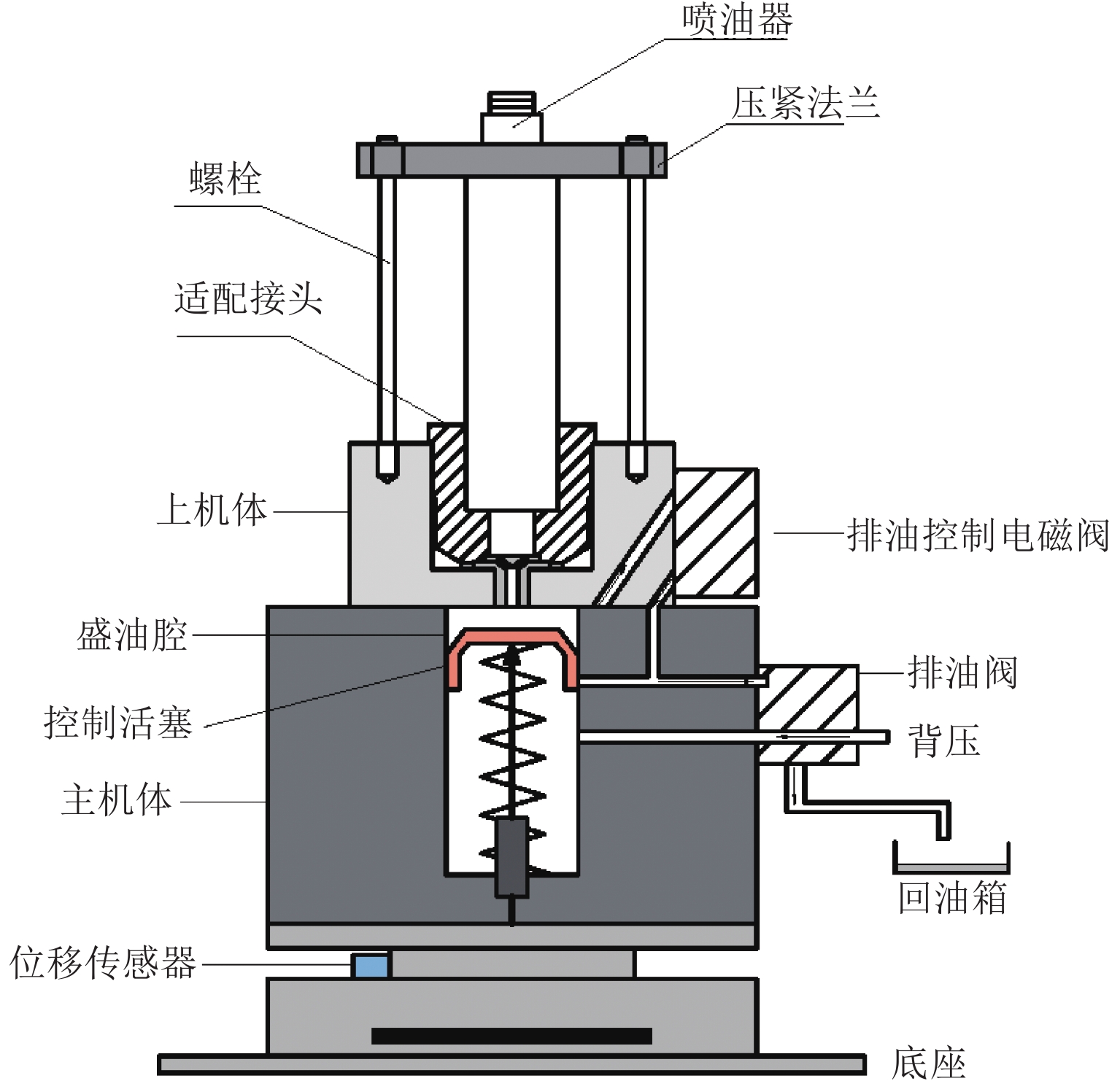
图2所示为本文建立的基于动量法的喷油器喷孔喷油规律测量装置. 该测量装置包括高压共轨喷油系统、高压共轨喷油系统控制台、共轨喷油器、实验柜、瞬态力传感器、油雾过滤器、数据实时采集系统、实验柜.

图 2 喷孔喷油规律测量装置

Figure 2. Measuring device for injection pattern of nozzle hole

下载: 全尺寸图片幻灯片

图3为法国EFS公司的EMI 2型单次喷射仪，在进行喷油规律测量时，首先将喷油器安装在单次喷射测量仪机械项部，调整背压关闭排油阀，然后向底部安装有可上下移动活塞的密封盛油腔内喷射燃油，从而导致控制活塞产生位移. 通过位移传感器将位移以电压信号输出，在对电压信号进行处理后，便可以得到相应位移下的循环喷油量以及相应的喷油规律曲线. EMI 2型单次喷射仪是EFS公司较为成熟的商业化测试仪器，测量误差小于满量程的± 0.1%，广泛应用在喷油规律测量的研究中^[14-15].

图 3 EMI 2型商业单次喷射仪

Figure 3. EMI 2 commercial single injection instrument

下载: 全尺寸图片幻灯片

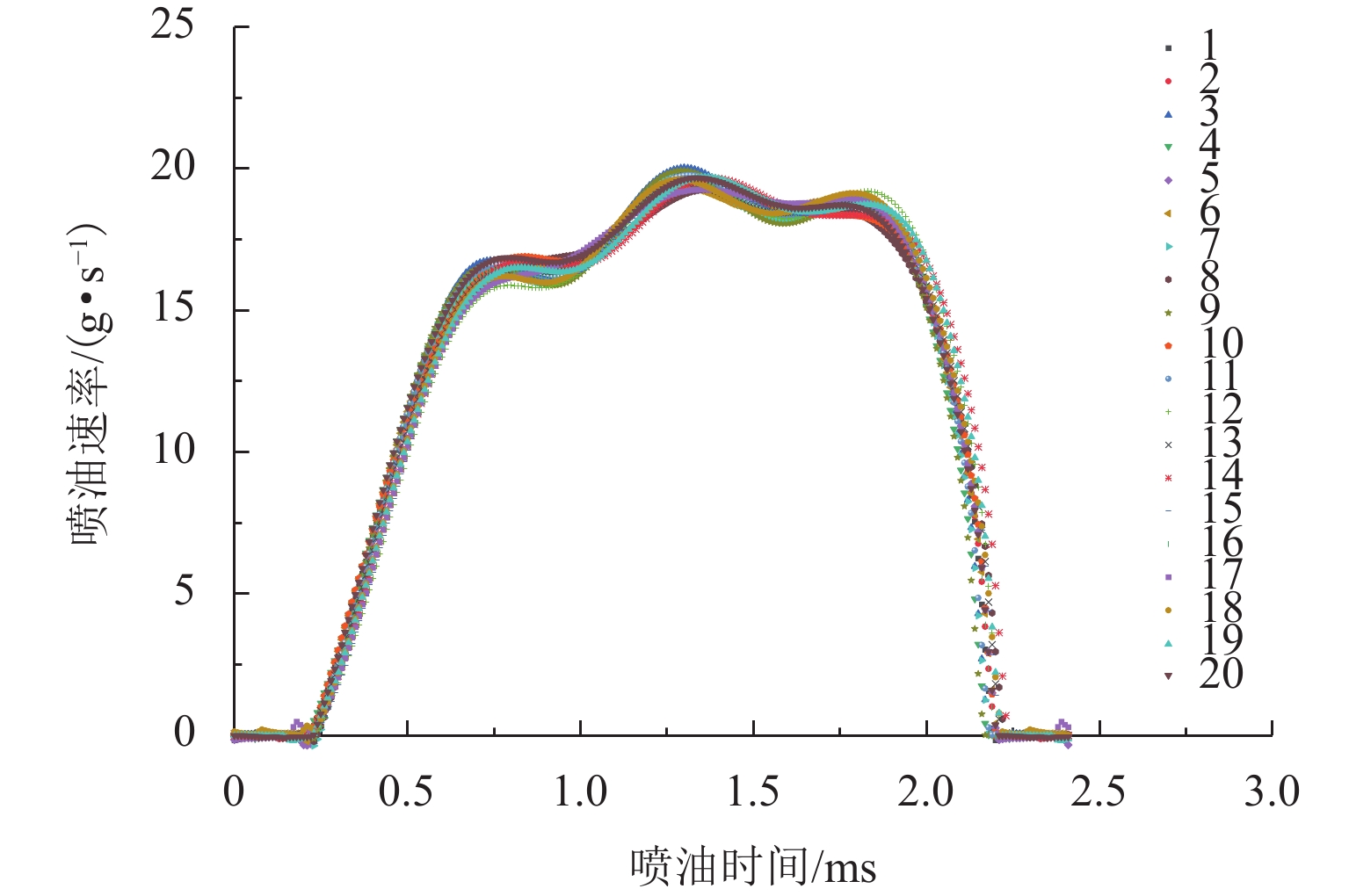
图4为所开发的实验装置在喷油压力100 MPa和喷油脉宽1.5 ms的条件下重复进行20次喷射的喷油规律. 由图4可知：每次喷射过程中喷油规律曲线的变化趋势均相同，并且不同喷射次数下的波谷、波峰出现的时间也一致；从循环喷油量来看，第9次喷射的循环喷油量的最大为131.23 mg，最小的循环喷油量出现在第16次为122.87 mg，循环喷油量的最大测量误差为6.8%，本文所开发的实验装置具有较好的复现性.

图 4 喷油规律重复性误差分析

Figure 4. Repeatability error analysis of injection pattern

下载: 全尺寸图片幻灯片

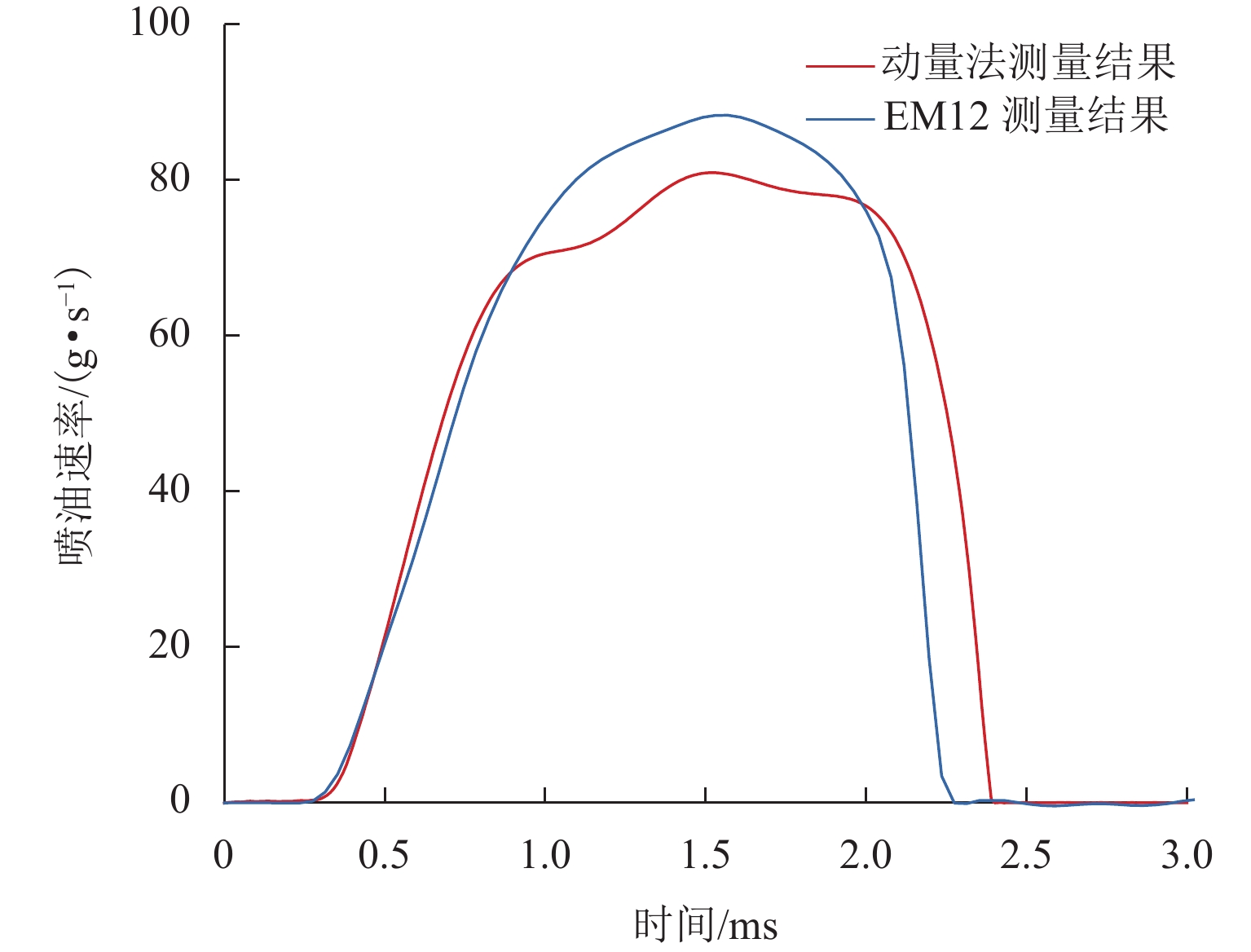
图5所示为喷油压力100 MPa和喷油脉宽1.5 ms，本文开发的测量装置和EFS公司的EMI 2型商业单次喷射仪在重复进行20次试验下测量结果均值的对比. 从图5看到：在喷油速率上升阶段，本文测量装置的测量结果和EMI 2测量结果的一致性较好，但在最大喷油速率上存在一定的差异，本文测量的最大值为80.96 g/s，而EIM2测量值为88.34 g/s，两者之间的偏差为8.4%，满足不超过10%的工程误差的要求^[16]；在喷油速率下降阶段，本文测量的喷油速率下降较EMI 2更缓，喷油结束时刻测量值晚于EMI 2测量值. Payri等^[17]在采用动量法进行喷油规律测量时同样出现了上述喷油速率下降段延迟的现象，在采用动量法进行喷油规律测量时，喷孔和传感器之间存在一定的距离，在针阀关闭后会有一部分油束需要一定的时间才能到达传感器表面，造成了基于动量法测量出现的后端喷油规律变缓的现象，但这对喷油量几乎没有影响.

图 5 研发的测量装置和EFS喷射仪测量结果对比

Figure 5. Comparison of measurement results between developed measuring device and EFS injector

下载: 全尺寸图片幻灯片

实际上，循环喷油量是保证柴油机所需动力性能的重要参数. 从循环喷油量的测量结果来看，本文测量值为126.38 mg，EMI2测量值为126.53 mg，两者的相对测量偏差仅为0.12%. 因此，从喷油规律曲线和循环喷油量可以看到，本文研发的基于动量法的喷孔喷油规律测量装置具有较高的测量准确性，可以采用该测量装置进行后续喷油特性测量研究.

4. 结果与讨论

4.1 各喷孔喷油差异性实验研究

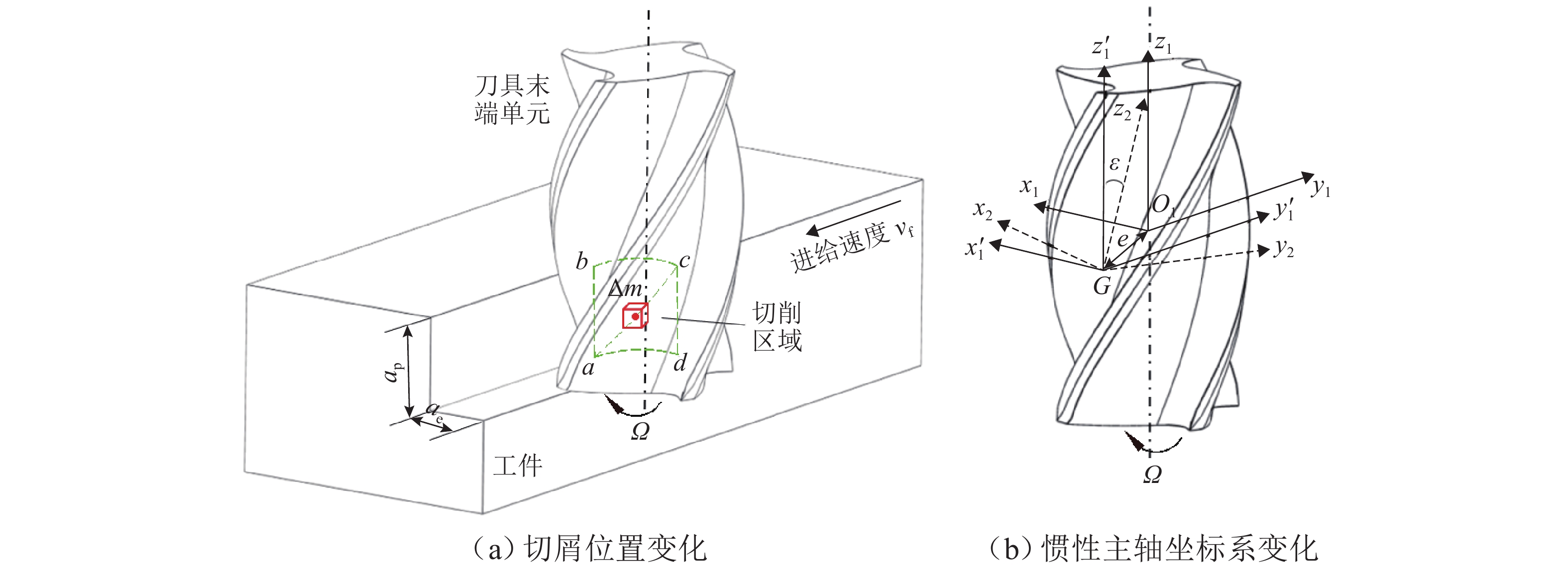
图6为本文采用的Bosch多孔喷油器结构示意，在喷嘴上均匀分布4个直径为0.3 mm，水平夹角为20° 的喷孔，针阀位于喷油器的中心，通过电磁阀控制针阀的起落改变燃油的流通面积.

图 6 喷油器结构示意

Figure 6. Structure of injector

下载: 全尺寸图片幻灯片

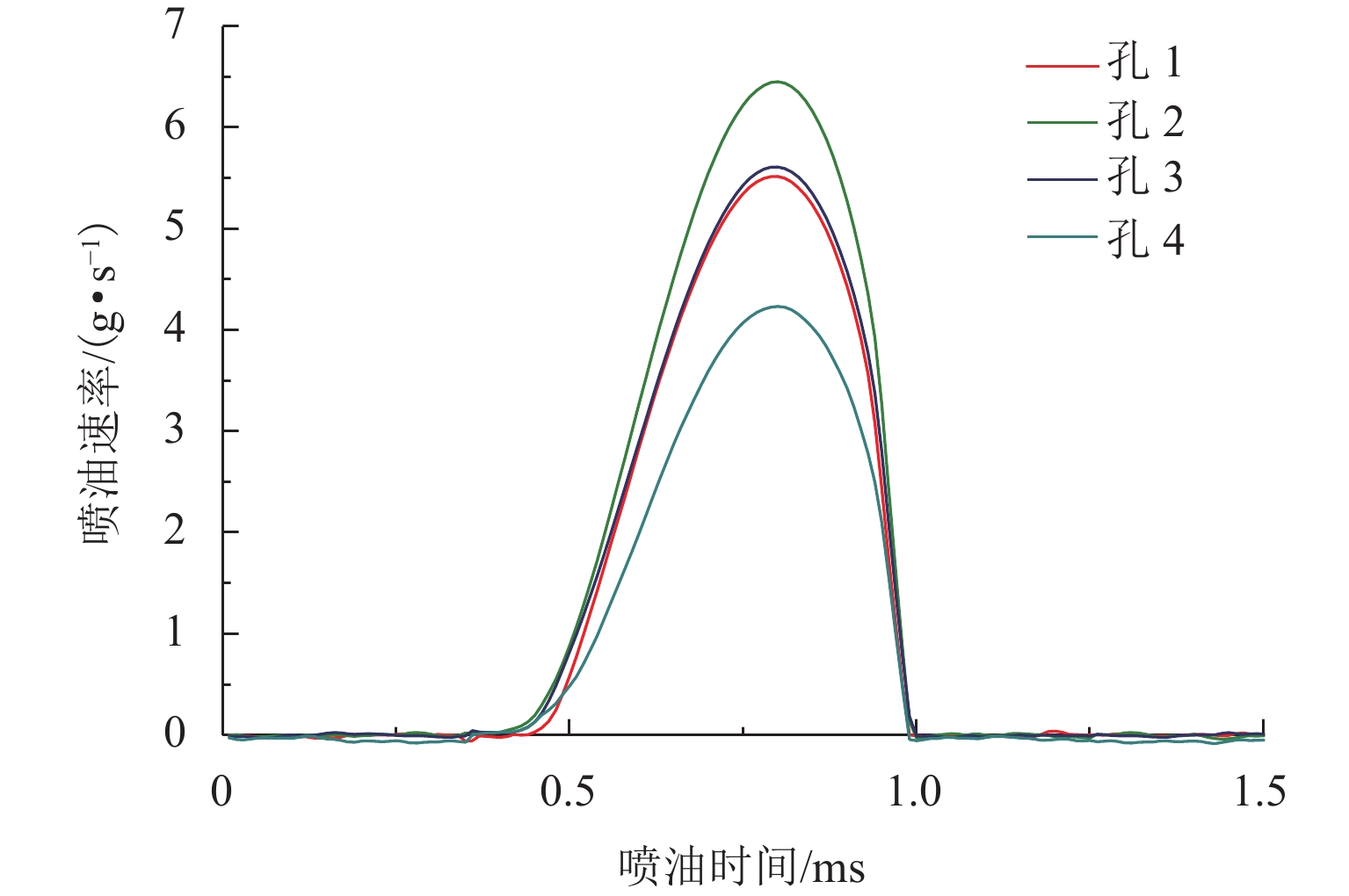
喷油压力60 MPa，喷油脉宽0.5 ms时各喷孔喷油速率实验结果对比如图7. 从图7观察到：喷孔间喷油速率差异较大，孔4喷油速率的峰值最小，孔2喷油速率的峰值最大，而孔1 （1.84 mg）和孔3（1.91 mg）喷油速率曲线几乎重合在一起；从循环喷油量角度分析发现，孔4喷油量为1.40 mg，是循环喷油量最小的，而孔2的循环喷油量为2.18 mg，为最大的，孔4和孔2循环喷油量的测量偏差达到55.71 %. 孔4和孔2喷油规律曲线显著差异的原因可能是针阀在抬升和落座过程中发生了向孔4方面的偏心，从而使得孔4实际的喷孔流通面积减小.

图 7 各喷孔喷油速率实验结果对比

Figure 7. Comparison of experimental results of injection rate of each nozzle hole

下载: 全尺寸图片幻灯片

4.2 低喷油压力下喷油稳定性实验研究

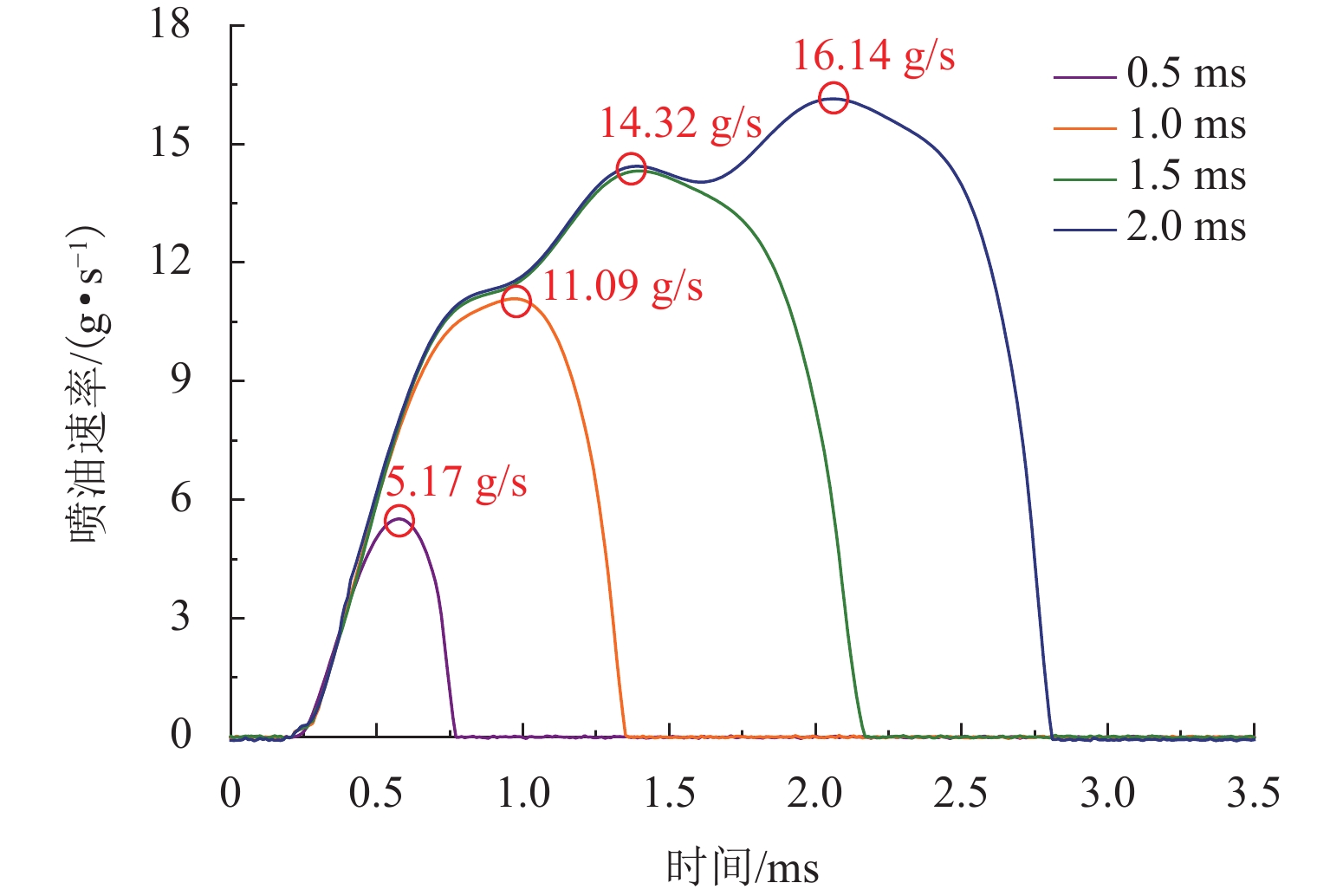
通过3.1部分研究发现，喷油压力和喷油脉宽共同作用会影响喷孔喷油的稳定性. 图8 为低喷油压力下（60 MPa）喷油速率随喷油脉宽的变化. 从图8看到：在脉宽为0.5 ms时，由于针阀没有完全打开而出现小喷油量；随着喷油脉宽从0.5 ms增加到2.0 ms过程中，喷油速率最大值不断增大，喷油速率曲线出现波动，这是由于针阀运动导致了高压共轨喷油器内产生了明显的压力波动，而压力波动又反过来影响喷油速率曲线的波动.

图 8 在低喷油压力下喷油速率随喷油脉宽的变化

Figure 8. Variation of injection rate with injection pulse width under low injection pressure

下载: 全尺寸图片幻灯片

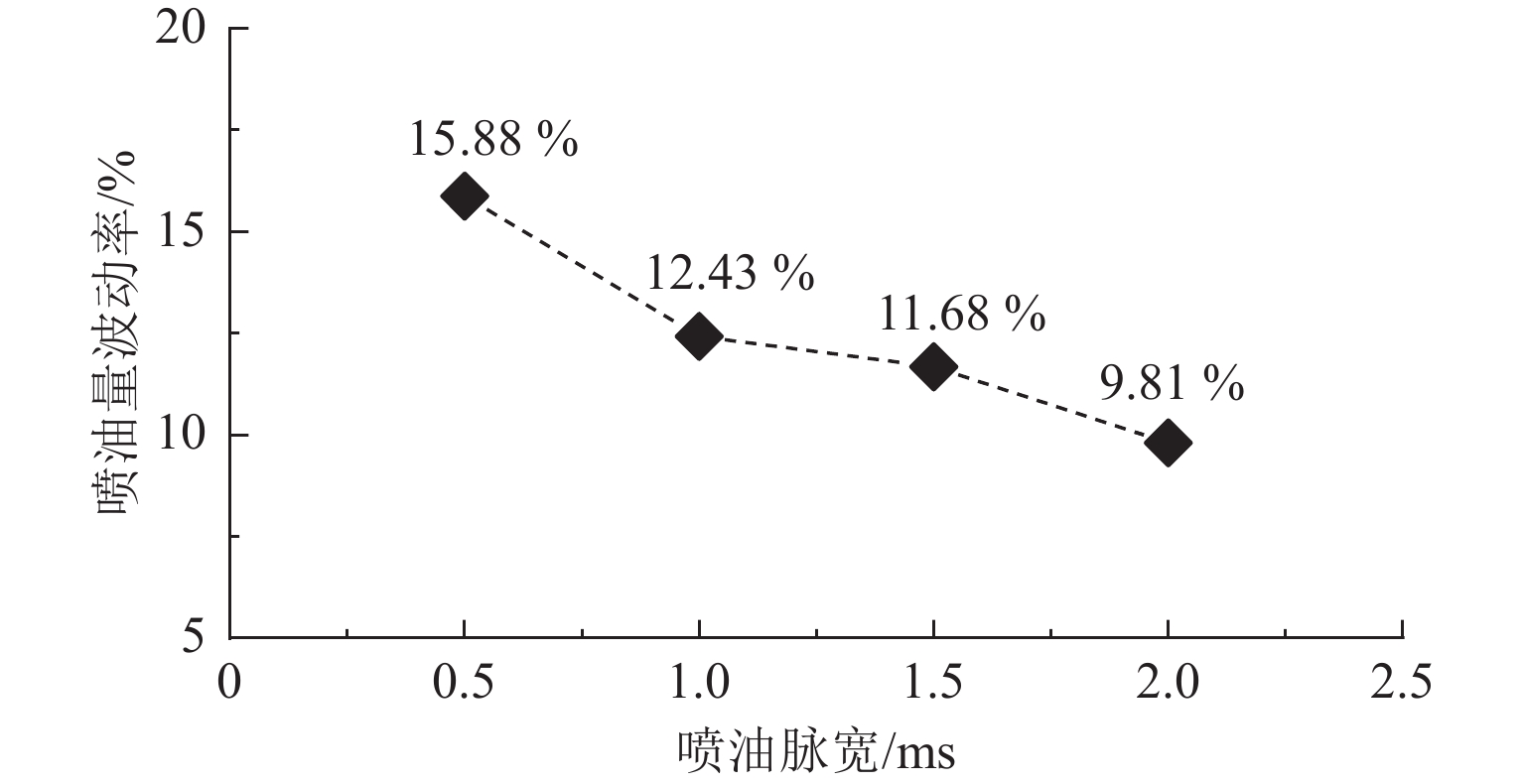
为了更清楚获得不同脉宽下喷孔喷油量波动率，针对孔3，采用式（4）计算50次喷射实验下喷油量波动率，如图9. 从图9看到：随着喷油脉宽的增加，喷孔喷油量波动率从15.88%降低9.81%，降幅为37.97%. 这个现象的原因在于，对于60 MPa小喷油压力，本文共轨喷油器理论上可实现的最大喷油速率为15.41 g/s，在喷油脉宽＜2 ms条件下，针阀并没有完全打开，此时，针阀抬起后便落座，未达到最大限位，使得针阀及针阀座之间的燃油流动成为主导，针阀运动不稳定引起了较大的喷油量波动率；随着针阀完全打开，针阀运动的不稳定性降低，喷油量波动率随着喷油脉宽的增加而减小.

图 9 低喷油压力下喷油量波动率随喷油脉宽的变化

Figure 9. Variation of fluctuation rate of injection with injection pulse width under low injection pressure

下载: 全尺寸图片幻灯片

4.3 高喷油压力下喷油稳定性实验研究

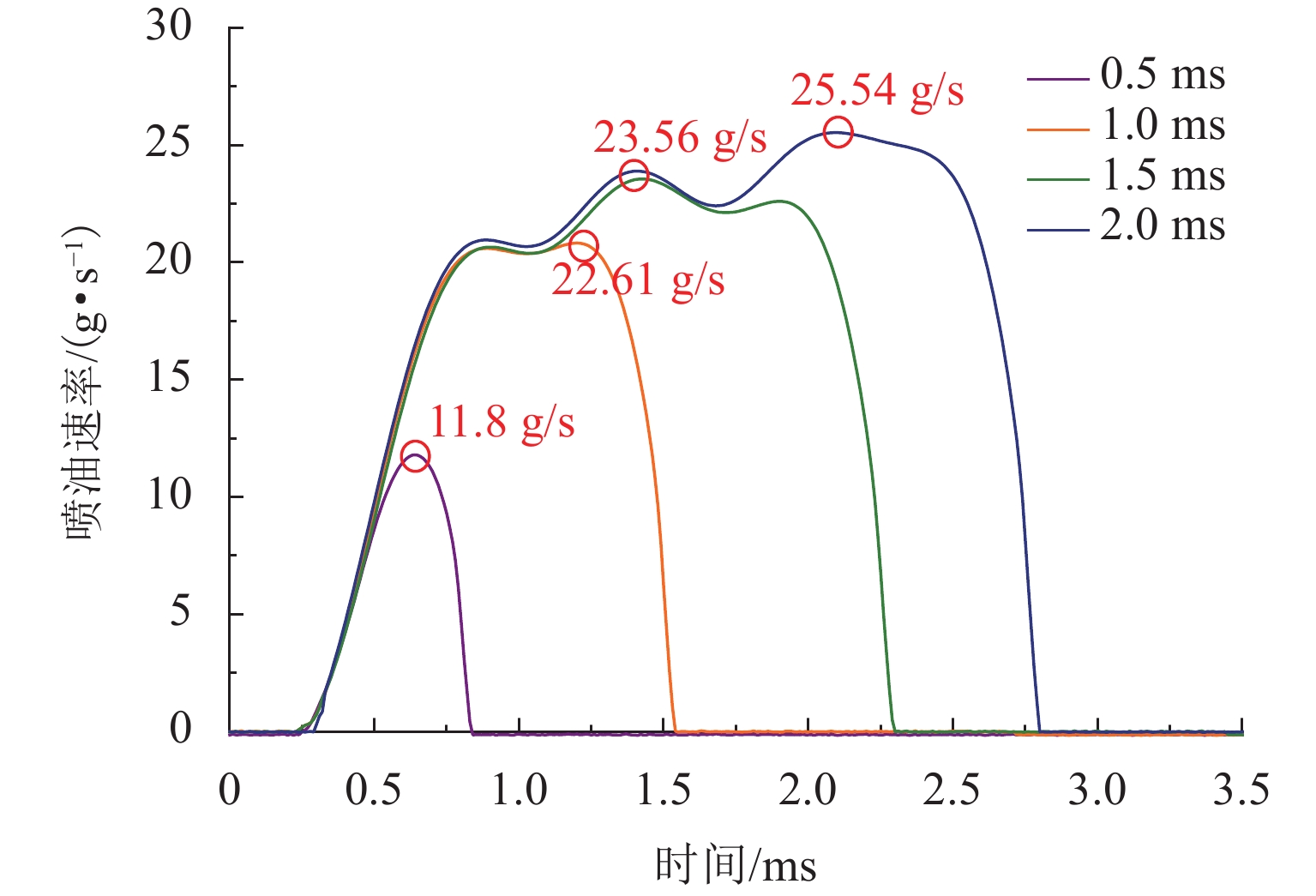
图10为高喷油压力下（120 MPa）喷油速率随喷油脉宽的变化. 从图10看到：当喷油脉宽为0.5 ms时，针阀并没有达到最大升程位置，而当喷油脉宽增加到1.0 ms时，喷油速率出现了稳定段，并且其最大喷油速率为22.61 g/s，和理论值22.23 g/s几乎一致，从而判断出喷油脉宽1.0 ms下针阀完全打开；随着喷油脉宽继续增加到2.0 ms，喷油速率中间平缓段变长，尽管存在一定的波动，主要是由于喷油诱发的压力波动导致.

图 10 高喷油压力下喷油速率随喷油脉宽的变化

Figure 10. Variation of injection rate with injection pulse width under high injection pressure

下载: 全尺寸图片幻灯片

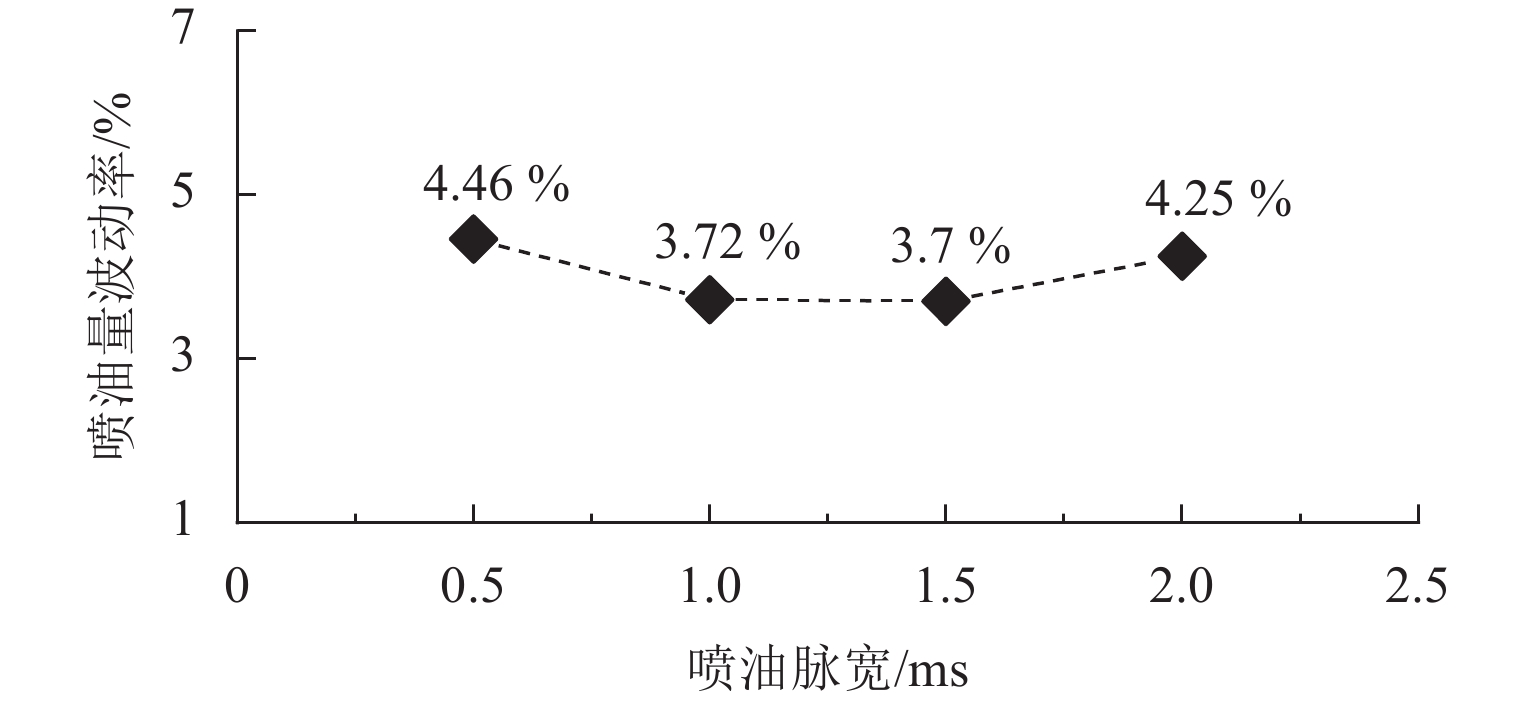
与图9所示的低喷油压力下脉宽对喷油量波动率的影响相比，从图11看到：在更高的喷油压力下，不同脉宽的喷油量波动率均降低，且随着脉宽的增加，喷油量波动率降低变缓. 这是因为，随着喷油压力的上升，相同的脉宽下针阀更容易达到最大升程. 结合图10可以观察到：在120 MPa喷油压力下，脉宽大于1.0 ms时，喷油速率出现了稳定段，对应的喷油波动率也降低；由于压力波动的影响，喷油量的循环波动率也产生波动，因此才出现了在脉宽为2.0 ms时循环波动率增大的现象，但仅增加了1%. 再次说明，针阀无法实现最大升程是高压共轨燃油喷射系统喷射不稳定性的主要原因.

图 11 在高喷油压力下喷油速率随喷油脉宽的变化

Figure 11. Variation of injection rate with injection pulse width under high injection pressure

下载: 全尺寸图片幻灯片

5. 结　论

1）基于动量法开发了共轨喷油器喷孔喷油规律测量装置，与商业单次喷射仪测量结果进行了对比，在最高喷油速率方面，两者测量偏差小于10%；在循环喷油量方面，两者测量偏差为0.12%，证明了本文研发的测量装置测量的准确性.

2）在低喷油压力条件下，随着喷油脉宽从0.5 ms增加到2.0 ms，喷孔喷油量波动率随之降低，在上述低喷油压力和脉宽条件下，针阀无法完全打开，针阀—针阀座之间燃油的不稳定流动成为影响喷油量波动的主要因素.

3）更高的喷油压力下，喷油脉宽相同时，针阀更容易达到最大升程，对喷孔喷油量波动起到决定作用的是喷孔参数. 因此，在0.5～2.0 ms喷油脉宽范围内喷孔喷油量波动率处于5%之内. 这说明，针阀无法实现最大升程是高压共轨系统燃油喷射不稳定性的主要原因.

图 1 磁悬浮铣削电主轴系统模型示意

Figure 1. System model of motorized spindle for magnetic suspension milling

下载: 全尺寸图片幻灯片

图 2 铣削过程示意

Figure 2. Milling process

下载: 全尺寸图片幻灯片

图 3 切屑质量变化示意

Figure 3. Chip quality change

下载: 全尺寸图片幻灯片

图 4 带有切屑的转动单元

Figure 4. Rotational unit with chips

下载: 全尺寸图片幻灯片

图 5 “主轴-刀具-切屑”变质量系统的坎贝尔图

Figure 5. Campbell diagram of “spindle–tool–chip” time-varying mass system

下载: 全尺寸图片幻灯片

图 6 切屑质量对“主轴-刀具-切屑”变质量系统临界转速的影响

Figure 6. Influence of chip quality on critical speed of “spindle–tool–chip” time-varying mass system

下载: 全尺寸图片幻灯片

图 7 前三阶模态振型图

Figure 7. The first three mode shapes

下载: 全尺寸图片幻灯片

图 8 磁悬浮电主轴-刀具-切屑系统振动响应

Figure 8. Vibration response of motorized spindle of magnetic suspension–tool–chip system

下载: 全尺寸图片幻灯片

图 9 磁悬浮电主轴-刀具-切屑系统切削加工点的振动响应

Figure 9. Vibration response of motorized spindle of magnetic suspension–tool–chip system at cutting point

下载: 全尺寸图片幻灯片

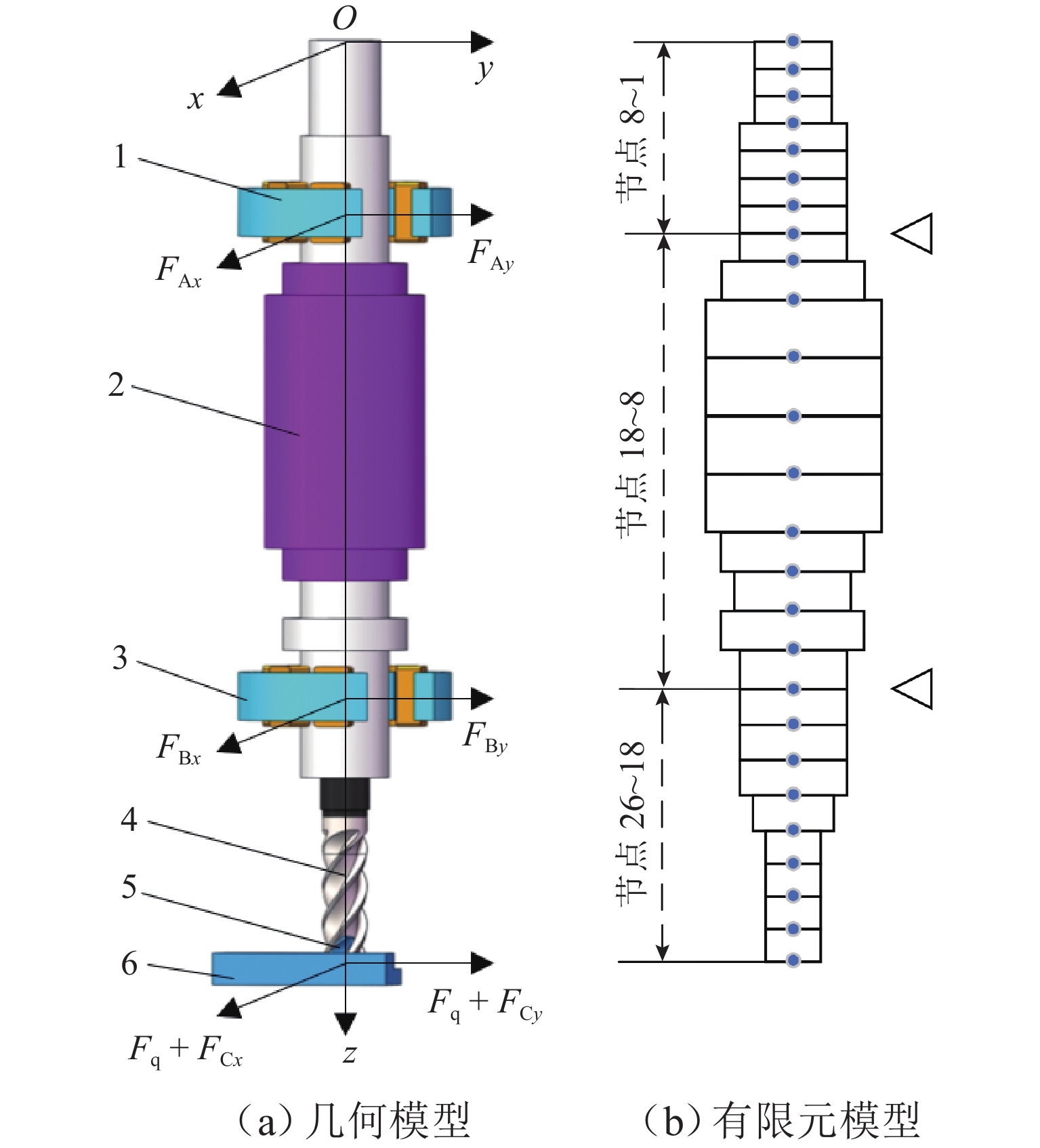
表 1 磁悬浮电主轴-刀具系统的物理几何参数

Table 1. Physical geometric parameters for motorized spindle of magnetic suspension–tool system

参数	数值
转子总长/mm	500
转子质量/kg	6.427
转子材料	30CrNiMo8
转子弹性模量/Pa	2 × 10¹¹
刀具总长/mm	80
刀具直径/mm	16
刀具材料	W6Mo5Cr4V2Co8
工作转速/（r•min⁻¹）	15000
磁轴承等效刚度/（N•m⁻¹）	−2 × 10⁷

下载: 导出CSV

参考文献(24)

[1]	熊万里,孙文彪,刘侃,等. 高速电主轴主动磁悬浮技术研究进展[J]. 机械工程学报,2021,57(13): 1-17. XIONG Wanli, SUN Wenbiao, LIU Kan, et al. Active magnetic bearing technology development in high-speed motorized spindles[J]. Journal of Mechanical Engineering, 2021, 57(13): 1-17.
[2]	朱润生,杨作兴,赵雷,等. 主动磁轴承电主轴的磨削试验[J]. 机械工程学报,2002,38(6): 134-138. ZHU Runsheng, YANG Zuoxing, ZHAO Lei, et al. Grinding experiment using electrospindle levitated with active magnetic bearings[J]. Journal of Mechanical Engineering, 2002, 38(6): 134-138.
[3]	钟志贤,祝长生. 主动磁轴承控制器对Jeffcott转子裂纹故障特征的影响[J]. 中国电机工程学报,2012,32(5): 105-110. ZHONG Zhixian, ZHU Changsheng. Effects of active magnetic bearing controller on fault characteristics of jeffcott cracked rotor[J]. Proceedings of the CSEE, 2012, 32(5): 105-110.
[4]	吴超,苏振中,张贤彪,等. 磁悬浮汽轮机多盘转子动力学建模与参数辨识[J]. 中国电机工程学报,2021,41(2): 442-451. WU Chao, SU Zhenzhong, ZHANG Xianbiao, et al. Multi-disc rotordynamic modeling and parameter identification of magnetic levitation turbine[J]. Proceedings of the CSEE, 2021, 41(2): 442-451.
[5]	LEI S L, PALAZZOLO A. Control of flexible rotor systems with active magnetic bearings[J]. Journal of Sound and Vibration, 2008, 314(1/2): 19-38.
[6]	KUNG H K, HUANG B W. Vibration in a cracked machine tool spindle with magnetic bearings[J]. The Open Mechanical Engineering Journal, 2008, 2(1): 32-39. doi: 10.2174/1874155X00802010032
[7]	BOUAZIZ A, BARKALLAH M, BOUAZIZ S, et al. Cutting parameters and vibrations analysis of magnetic bearing spindle in milling process[J]. Journal of Theoretical and Applied Mechanics, 2016, 54: 691-703.
[8]	ZHANG Y, ZHOU J, ZHANG Y B, et al. Modelling and vibration response of a magnetically suspended flexible rotor considering base motion[J]. Applied Mathematical Modelling, 2023, 118: 518-540. doi: 10.1016/j.apm.2023.01.020
[9]	陈小安,陈文曲,康辉民,等. 偏心电主轴动力学分析[J]. 重庆大学学报,2012,35(3): 26-32. CHEN Xiaoan, CHEN Wenqu, KANG Huimin, et al. Dynamic analysis of high speed motorized spindles with eccentrics[J]. Journal of Chongqing University, 2012, 35(3): 26-32.
[10]	陈小安,张朋,陆永亚,等. 计电磁不平衡拉力的高速电主轴转子偏心特性研究[J]. 振动与冲击,2014,33(2): 37-40. CHEN Xiaoan, ZHANG Peng, LU Yongya, et al. Dynamic characteristics of high-speed motorized spindles affected by rotor’s eccentric mass[J]. Journal of Vibration and Shock, 2014, 33(2): 37-40.
[11]	宋春生,尹睿,魏子航,等. 磁悬浮柔性转子系统解耦控制仿真[J]. 西南交通大学学报,2023,58(4): 761-772. SONG Chunsheng, YIN Rui, WEI Zihang, et al. Simulation on decoupling control of maglev flexible rotor system[J]. Journal of Southwest Jiaotong University, 2023, 58(4): 761-772.
[12]	王艺宇,周瑾,周扬,等. 考虑界面接触的磁悬浮转子高阶模态自激振动抑制研究[J]. 振动与冲击,2023,42(23): 29-40. WANG Yiyu, ZHOU Jin, ZHOU Yang, et al. Suppression of high order modal self-excited vibration of magnetic levitation rotor considering interface contact[J]. Journal of Vibration and Shock, 2023, 42(23): 29-40.
[13]	彭超英,高燕青,朱均. 纺织机械变质量转子的振动机理分析[J]. 机械科学与技术,1996(1): 60-64.
[14]	彭超英,陈瑞琪,朱均. 纺织机械变质量转子在不同支承下非线性自由振动研究[J]. 中国纺织大学学报,1993(3):1-7. PENG Chaoying, CHEN Ruiqi, ZHU Jun. Study on nonlinear free vibration of variable mass rotor of textile machinery under different supports[J]. Journal of China Textile University, 1993(3):1-7.
[15]	袁龙翔. 柔性工件车削颤振分析及试验验证[D]. 沈阳:东北大学,2020.
[16]	CAO Z, KANG H M, LIU H C, et al. Modeling and dynamic response of variable mass system of maglev turning electric spindle[J]. Nonlinear Dynamics, 2023, 111(1): 255-274. doi: 10.1007/s11071-022-07868-8
[17]	欧阳智海,康辉民,刘厚才,等. 磁悬浮铣削电主轴转子-刀具变质量不平衡系统动态特性研究[J]. 机械工程学报,2022,58(23): 306-320. OUYANG Zhihai, KANG Huimin, LIU Houcai, et al. Study on dynamic characteristics of magnetic suspension milling motorized spindle rotor-tool variable mass unbalance system[J]. Journal of Mechanical Engineering, 2022, 58(23): 306-320.
[18]	袁惠群. 转子动力学分析方法[M]. 北京:冶金工业出版社,2017.
[19]	MARTELLOTTI M E. An analysis of the milling process[J]. Journal of Fluids Engineering, 1941, 63(8): 677-695.
[20]	杨中宝. 基于切削图形的动态铣削力建模[D]. 天津:天津理工大学,2011.
[21]	洪杰,杨哲夫,孙博,等. 局部旋转惯性对转子系统动力特性的影响[J]. 航空动力学报,2022,37(4): 673-683. HONG Jie, YANG Zhefu, SUN Bo, et al. Influence of local rotary inertia on the dynamic properties of rotor systems[J]. Journal of Aerospace Power, 2022, 37(4): 673-683.
[22]	王亚辉,王剑华,任亚杰. 理论力学[M]. 北京:科学出版社,2020.
[23]	陈攀,康辉民,陈鹏,等. 主动磁悬浮电主轴最佳切削速度区间研究[J]. 机械科学与技术,2019,38(12): 1904-1909. CHEN Pan, KANG Huimin, CHEN Peng, et al. Research on optimal cutting speed range of active magnetic suspension electric spindle[J]. Mechanical Science and Technology for Aerospace Engineering, 2019, 38(12): 1904-1909.
[24]	张耀宸. 机械加工工艺设计实用手册[M]. 北京: 航空工业出版社,1993.