主动磁悬浮轴承非奇异快速终端滑模转子位置控制

巩磊; 何派; 石勇; 祝长生

doi:10.3969/j.issn.0258-2724.20240090

主动磁悬浮轴承非奇异快速终端滑模转子位置控制

doi: 10.3969/j.issn.0258-2724.20240090

巩磊^1,,
何派¹,
石勇¹,
祝长生²

1.
陕西科技大学电气与控制工程学院，陕西西安 710016
2.
浙江大学电气工程学院，浙江杭州 310027

基金项目: 国家自然科学基金项目（52407059）；陕西省自然科学基础研究计划（2023-JC-QN-0441）

详细信息

作者简介:
巩磊（1993—），男，讲师，研究方向为高速转子系统振动主动控制，E-mail：gong_lei@sust.edu.cn

中图分类号: TH133.3
计量
- 文章访问数: 125
- HTML全文浏览量: 109
- PDF下载量: 14
- 被引次数: 0
出版历程
- 收稿日期: 2024-02-27
- 修回日期: 2024-06-13
- 网络出版日期: 2024-11-15

Non-Singular Fast Terminal Sliding Mode Rotor Position Control of Active Magnetic Bearings

1.
School of Electrical and Control Engineering, Shaanxi University of Science & Technology, Xi’an 710016, China
2.
College of Electrical Engineering, Zhejiang University, Hangzhou 310027, China

摘要

摘要:
针对主动磁悬浮轴承转子位置控制中系统响应速度慢、抗干扰能力弱等问题，本文提出将非奇异快速终端滑模函数与改进超螺旋趋近律相结合的位置控制方法，以获得快速、精确的动态响应控制效果. 由于系统内扰和外扰的存在，需要对滑模趋近律增加常值切换增益以保证系统的强鲁棒性，但会使得系统抖振进一步变大，本文通过采用非线性扩张状态观测器对干扰进行实时观测并动态补偿，折衷了抖振与抗干扰性之间的矛盾；通过李雅普诺夫稳定性理论证明了所提方法的稳定性，并对提出的控制方法进行仿真与实验验证. 研究结果表明：与传统的滑模控制器相比，所设计的控制器具有更快的响应速度和更强的抖振抑制能力，转子达到目标位置的时间缩短了56.4%，系统动态性能得到改善；控制电流的平均值减小了68.5%，系统抑制抖振的能力增强，即算法具有较强的鲁棒性.
- 主动磁悬浮轴承 /
- 非奇异快速终端 /
- 位置控制 /
- 扩张状态观测器
Abstract:
In order to solve problems of slow system response speed and poor anti-interference ability in the position control of the rotor of active magnetic bearings (AMBs), a position control method combining the non-singular fast terminal sliding function with the improved super-twisting reaching law was proposed to obtain fast and accurate control effects of dynamic responses. In addition, due to internal and external interference in the system, constant switching gain was added to the sliding mode reaching law, so as to ensure the robustness of the system, but it could exacerbate the system chattering. Therefore, the interference was observed and compensated by a nonlinear extended state observer, which alleviated the contradiction between chattering and anti-interference. Then, the stability of the proposed method was proven Lyapunov stability theory, and the proposed control method was verified by simulation and experiment. The results show that compared with the traditional sliding mode controller, the designed controller has faster response speed and stronger chattering suppression ability, and the time for the rotor to reach the target position is shortened by 56.4%. The dynamic performance of the system is improved, and the average control current is reduced by 68.5%. The chattering suppression ability of the system is enhanced, indicating that the proposed algorithm has strong robustness.
- active magnetic bearing /
- non-singular fast terminal /
- position control /
- extended state observer

HTML全文

在大功率高转速旋转机械系统中，主动磁悬浮轴承（AMBs）具有无摩擦、无润滑等优点，使得其得到了广泛的应用^[1-4]. 然而，如何控制AMBs转子稳定悬浮于期望位置一直以来是一个重要问题. AMBs是一个典型的非线性系统，一般采用局部线性化的方法进行建模^[5]，进而应用线性控制或非线性控制对其进行精确控制^[6]. 工程上一般采用PID控制^[7-8]，但AMBs在运行中会受到一些扰动，同时工况的改变会使得PID控制的鲁棒性大幅减弱. 因此，需要控制器自身具有较强的鲁棒性以克服AMBs系统的内扰和外扰，从而有效地提高其可靠性.

众多鲁棒控制算法中，滑模控制（SMC）具有适应性强、鲁棒性好、对未知参数和干扰不敏感、易于实现等优点，被广泛应用于包括AMBs在内的各种非线性系统. 然而，简单的滑模控制采用线性滑模函数，系统误差通常只能缓慢地渐近收敛，进而有学者采用基于动态非线性滑模函数的终端滑模控制方法，可以使得系统误差在有限的时间内实现快速收敛^[9]. 文献[10]中提出的双滑模控制器用于对开关磁阻电机进行调速；文献[11]针对伺服电机系统设计了一种新的连续终端滑模控制器，能够有效提高系统的鲁棒性；文献[12]针对永磁直线同步电动机位置控制问题，采用非奇异快速终端滑模，从而使系统获得快速、精确的跟踪性能；文献[13]设计了滑模自抗扰控制器，实现了对AMBs的各自由度的解耦，减小了AMBs在高速运转下锥动对控制效果的影响；文献[14]在磁悬浮球系统中将自适应控制与终端滑模控制结合，以此来减小系统抖振，改善悬浮系统的动态性能；文献[15]在磁悬浮球系统中将广义比例积分观测器与终端滑模控制结合，避免切换函数增益过大，有效地减小了系统抖振.

除了对滑模函数的设计，趋近律也需要进行优化，传统趋近律的切换增益为常数，系统抖振的大小与切换增益的大小有关. 超螺旋趋近律把切换增益改为与系统状态有关的幂函数，同时还利用积分函数对切换函数进行处理. 因此，超螺旋趋近律能够使系统状态在滑模面附近平滑且切换增益较小，减小系统抖振^[16-17]. WANG等^[18]将超螺旋趋近律与非奇异快速终端滑模相结合（SNFTSMC），以实现减小抖振并加快系统误差收敛.

在AMBs系统中，传感器跳动、电磁场波动等外部因素经常会导致AMBs转子位置控制的精度受到影响，而且AMBs系统在建模过程中对系统做了线性化，也导致AMBs数学模型含有未建模部分. 虽然采用SNFTSMC能够抑制未建模部分和外部扰动带来的影响，但这需要增大切换增益，又会造成系统抖振. 因此，SNFTSMC在抑制抖振和扰动之间存在一定的矛盾. 此时，将未建模部分与外部扰动看作一个集总干扰，利用扩张状态感测器（ESO）对集总干扰进行观测，然后补偿给系统，这种方法在伺服系统中已经得到了广泛的应用^[19-20]. 通常，线性ESO（LESO）参数易于整定，而非线性ESO（NESO）收敛精度高、鲁棒性强^[21-22].

因此，针对主动磁悬浮轴承转子位置控制中存在响应速度慢、抗干扰能力弱2个问题，本文采用SNFTSMC作为控制算法，并通过ESO对集总干扰观测并补偿到系统中. 由于LESO对于AMBs非线性系统观测效果较差，因此，本文采用了NESO观测器. 根据李雅普诺夫稳定理论证明了所提方法的稳定性，并通过仿真和实验验证了系统具有强的鲁棒性以及低抖振性能.

1. 单自由度AMBs系统模型

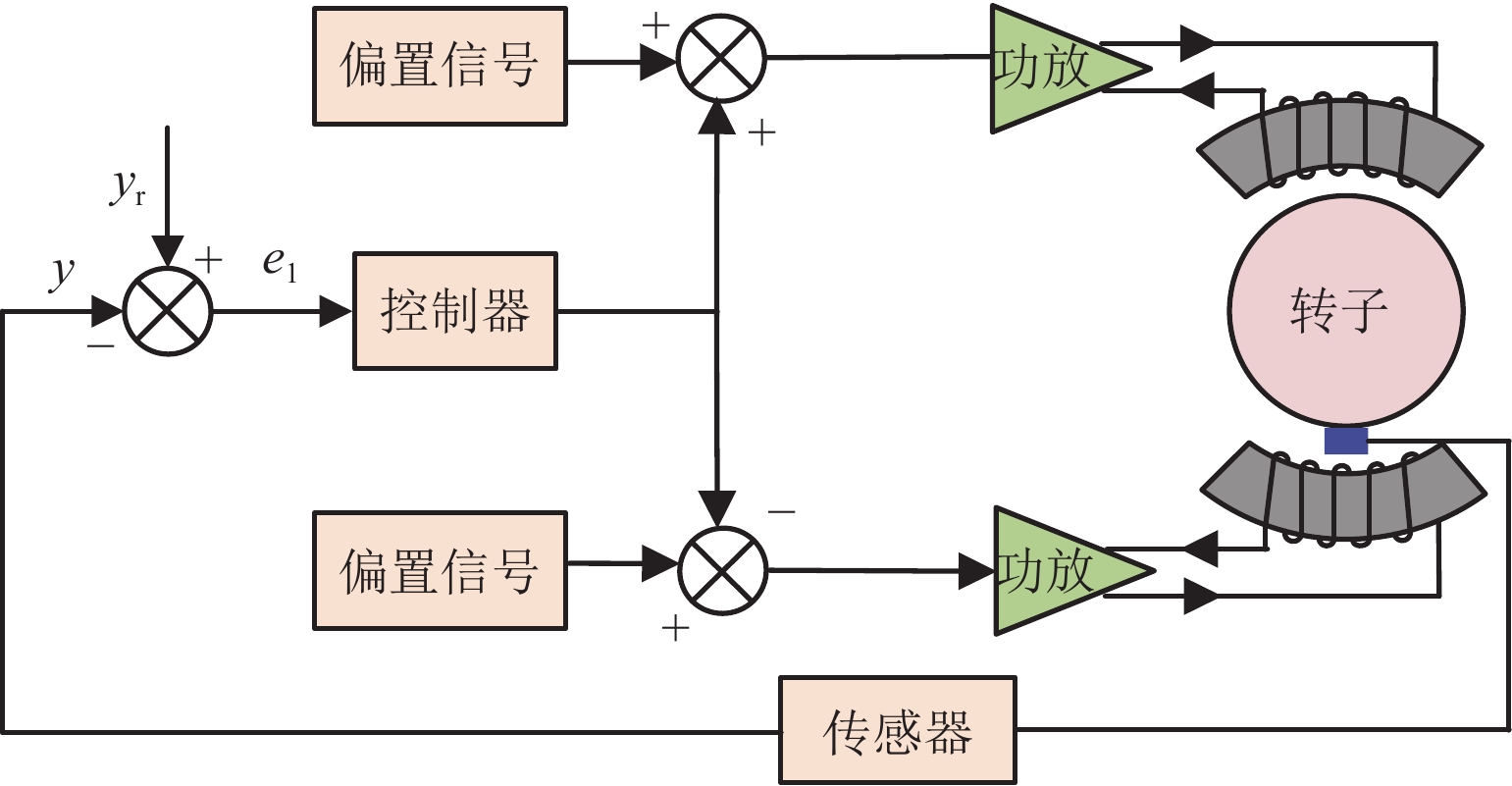
以径向单自由度的AMBs为研究对象，研究转子起浮运动. 单自由度AMBs系统通过传感器获得转子实际位移y；实际位移y与期望位置y_r的误差e₁输入到控制器中，控制器进行运算得到控制信号；之后，在与偏置信号进行差分；最后，将差分后的信号输送到功放器中产生相应的电流，电流被送到电磁铁线圈中，电磁铁产生吸力将转子吸引到期望位置. 具体工作原理如图1.

图 1 单自由度AMBs系统原理

Figure 1. Principle of single-degree-of-freedom AMB system

下载: 全尺寸图片幻灯片

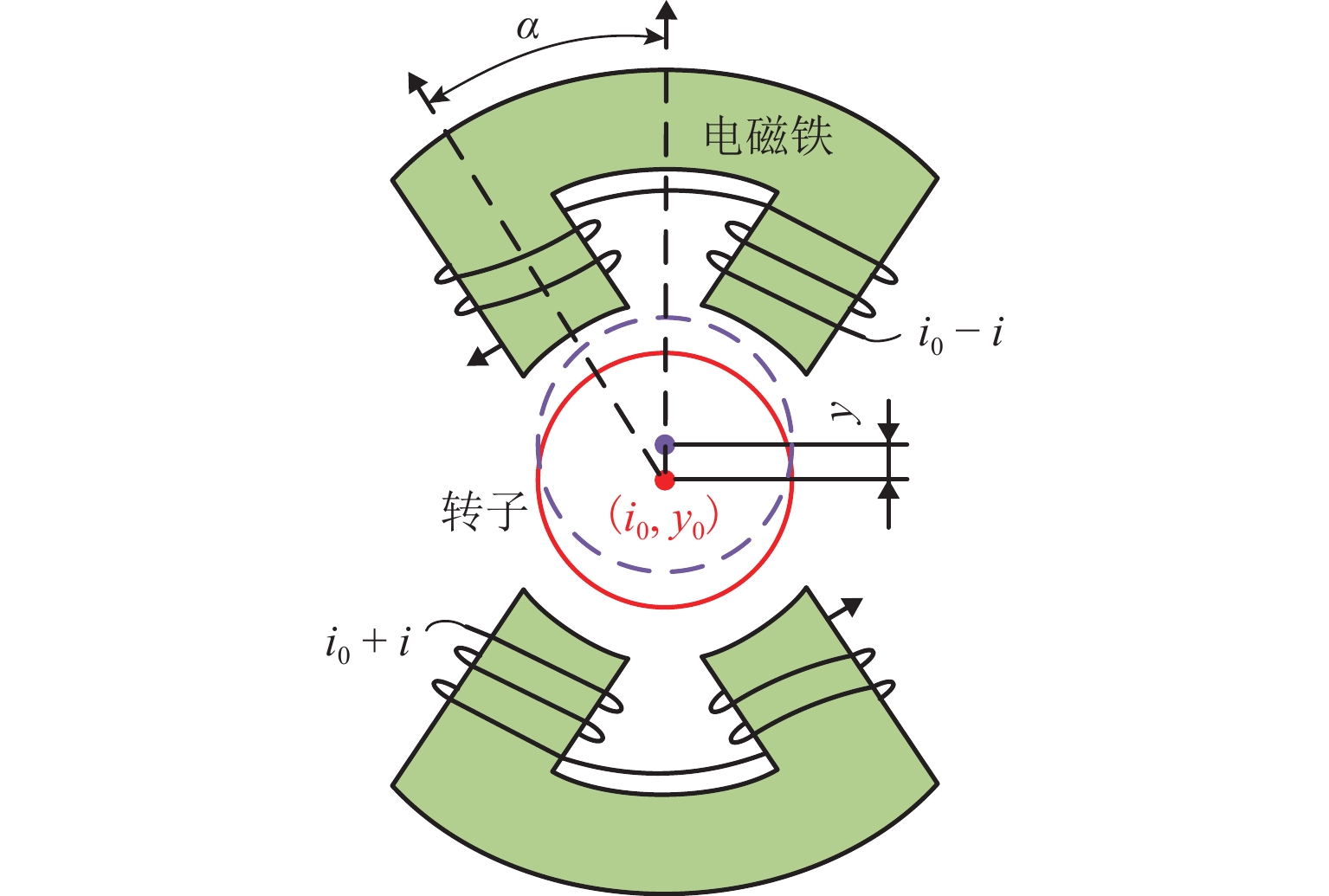
AMBs采用8级C型结构，根据麦克斯韦公式，2个磁极作用在转子上的电磁力为

$f = {\mu _0}{n^2}{i^2}A\cos\; \alpha /{l^2},$

(1)

式中：i为线圈通入的电流，l为气隙长度，A为单个磁极截面积，μ₀为真空磁导率，α为磁极之间夹角的一半，n为线圈匝数.

电磁铁对转子的控制方式为差动控制，在垂直方向上以（i₀，y₀）作为参考点，如图2. 当转子向上运动的位移为y时，转子与上方电磁铁之间的气隙间距变成y₀−y，则上方电磁铁线圈输入的工作电流为i₀−i；转子与下方电磁铁之间的气隙间距变成y₀+y，下方电磁铁线圈输入的工作电流为i₀+i，此时，在垂直方向上的合力为

图 2 差动控制原理图

Figure 2. Principle of differential control

下载: 全尺寸图片幻灯片

$f(i,y) = {\mu _0}A{n^2}\left[ {{{\left( {\frac{{{i_0} - i}}{{{y_0} - y}}} \right)}^2} - {{\left( {\frac{{{i_0} + i}}{{{y_0} + y}}} \right)}^2}} \right]\cos\; \alpha.$

(2)

式（2）中将y、i作为参考点（i₀，y₀）的邻域，在参考点（i₀，y₀）处对f（i，y）进行泰勒展开，如式（3）.

$f(i,y) = {k_{\mathrm{i}}}i + {k_{\mathrm{s}}}y + {f_{\mathrm{R}}},$

(3)

式中：f_R为高次项部分（也称未建模部分）；k_i、k_s分别为电流刚度系数和位移刚度系数，如式（4）、（5）.

${\begin{array}{*{20}{l}} {{k_{\mathrm{i}}} = 4{\mu _0}{N^2} {i_0}A\cos\; \alpha/y_0^2}, \\ {{k_{\mathrm{s}}} = 4{\mu _0}{N^2}i_0^2 A\cos\; \alpha /y_0^3}. \end{array}}$

(4)

将重力mg、未知扰动f_d都考虑到系统中，则系统的状态方程为

$\left\{ {\begin{array}{*{20}{l}} {y = {y_1}}, \\ {{{\dot y}_1} = {y_2}}, \\ {{{\dot y}_2} = {b_0}i + {a_0}{y_1} + d} , \end{array}} \right.$

(5)

式中： a₀为位移增益，a₀=k_i/m；b₀为位移增益，b₀=k_s/m；d为集总干扰，d=（f_R + f_d−mg）/m.

2. SNFTSMC设计

2.1 SNFTSMC设计

基于磁悬浮转子系统的数学模型，设计了非奇异快速终端滑模函数结构，具体表达式如式（6），相应结构如图3.

图 3 非奇异快速终端滑模函数结构

Figure 3. Structure of non-singular fast terminal sliding mode function

下载: 全尺寸图片幻灯片

$s = {e_1} + {k_1}{\left| {{e_1}} \right|^a}{\mathrm{sign}}({e_1}) + {k_2}{\left| {{e_2}} \right|^b}{\mathrm{sign}}({e_2}),$

(6)

式中：k₁、k₂、a、b为调节系数，k₁>0，k₂>0，1<b<2，a>b；e₁=y₁−y_r，e₂=$ {\dot y_1} - {\dot y_r} $.

滑模面为滑模函数s=0，令式（6）为0，得到

$0 = {e_1} + {k_1}{\left| {{e_1}} \right|^a}{\mathrm{sign}}({e_1}) + {k_2}{\left| {{e_2}} \right|^b}{\mathrm{sign}}({e_2}).$

(7)

设误差e₁从初始值e₁（0）收敛到0所用的时间为t_f，对式（7）进行求解，得到t_f的解为^[11]

$\begin{split} & {t_{\mathrm{f}}} = \int_0^{\left| {{e_1}(0)} \right|} {\frac{{k_2^{1/b}}}{{{{({e_1} + {k_1}{x^a})}^{1/b}}}}} {\mathrm{d}}{e_1} = \frac{{b{{\left| {{e_1}(0)} \right|}^{1 - 1/b}}}}{{{k_1}(b - 1)}}\times \\ &\quad {{F}}\left(\frac{1}{b}{\text{,}}\frac{{b - 1}}{{(a - 1)b}}{\text{;1 + }}\frac{{b - 1}}{{(a - 1)b}}; - {k_1}{\left| {{e_1}(0)} \right|^{a - 1}}\right) , \end{split}$

(8)

式中：F（·）为高斯几何函数.

对式（6）求导为

$\dot s = {e_2} + a{k_1}{\left| {{e_1}} \right|^{a - 1}}{e_2} + b{k_2}{\left| {{e_2}} \right|^{b - 1}}{\dot e_2}.$

(9)

忽略式（5）中集总干扰d，$\dot s $=0，可以得到等效控制器为

${i_{{\mathrm{eq}}}} = \frac{1}{{{b_0}}}\left({\ddot y_{\mathrm{r}}} - {a_0}{y_1} - \frac{1}{{b{k_2}}}{\left| {{e_2}} \right|^{2 - b}}(1 + a{k_1}{\left| {{e_1}} \right|^{a - 1}}){\mathrm{sign}}({e_2})\right).$

(10)

为了加快趋近速度和减小控制过程中出现的抖振，采用超螺旋趋近律，具体表达式如式（11），其结构如图4.

图 4 超螺旋趋近律结构

Figure 4. Structure of super-twisting reaching law

下载: 全尺寸图片幻灯片

超螺旋趋近律的具体表达式为

$\dot s = - {k_3}{\left| s \right|^c}{\mathrm{sign}}(s) - {k_4}\int {{\mathrm{sign}}(s){\mathrm{d}}t} ,$

(11)

式中：k₃、k₄、c为调节系数，k₃>0，k₄>0，0<c<1.

滑模函数s距离滑模面s=0较远时，$ - {k_3}{\left| s \right|^c} $值较大，滑模函数s以较大的速度靠近滑模面s=0；滑模函数s距离滑模面s=0较小时，$ - {k_3}{\left| s \right|^c}{\mathrm{sign}}(s) $值较小，滑模函数s以较小的速度靠近滑模面s=0. 滑模函数中sign函数的切换增益($ - {k_3}{\left| s \right|^c} $)的大小决定抖振剧烈程度，采用超螺旋趋近律既能削弱抖振又能加快系统收敛.

为了能够进一步加快滑模函数s到达滑模面s=0的速度及削弱抖振，可采用式（12）所示的趋近律.

$\left\{ {\begin{array}{*{20}{l}} {\dot s = - {k_3}{{\left| s \right|}^g}{\mathrm{sign}}(s) - {k_4}\int {{\mathrm{sign}}(s){\mathrm{d}}t} }, \\ {g = \gamma - \lambda {e^{ - \eta \left| {{e_1}} \right|}}} , \end{array}} \right.$

(12)

式中：γ、λ、η均为可调系数，g为关于γ、λ、η的指数函数，γ>1，0<λ，η>0.

由式（12）可知，随着e₁从初值e₁（0）衰减到0，g从最初的较大值γ−λ$ {{\mathrm{e}}^{ - \eta \left| {{e_1}(0)} \right|}} $衰减到γ−λ，在这个过程中$ - {k_3}{\left| s \right|^g} $能够以更快的速度从较大值衰减到0，从而加快滑模函数s到达滑模面s=0的速度和减小抖振.

因此，定义AMBs转子系统的切换控制器为

${i_{{\mathrm{sw}}}} = \frac{1}{{{b_0}}}\left[ - {k_3}{\left| s \right|^g}{\mathrm{sign}}(s) - {k_4}\int {{\mathrm{sign}}(s){\mathrm{d}}t} \right].$

(13)

考虑到系统存在集总干扰，总的控制器为

${i_{\mathrm{c}}} = {i_{{\mathrm{eq}}}} + {i_{{\mathrm{sw}}}} - \frac{1}{{{b_0}}}M{\mathrm{sign}}(s),$

(14)

式中：M为集总干扰d的上界，即$ d \leqslant \left| M \right| $.

2.2 稳定性分析

为了证明SNFTSMC控制器的稳定性，构造李雅普诺夫函数为

$V(s) = \frac{1}{2}{s^2},$

(15)

$\begin{split} & \dot V(s) = s\dot s = s[{e_2} + a{k_1}{\left| {{e_1}} \right|^{a - 1}}{e_2} + b{k_2}{\left| {{e_2}} \right|^{b - 1}}{{\dot e}_2}] = s[{e_2} + a{k_1}{\left| {{e_1}} \right|^{a - 1}}{e_2} + b{k_2}{\left| {{e_2}} \right|^{b - 1}}({b_0}{i_{\mathrm{c}}} + {a_0}{y_1} + d - {{\ddot y}_r})] = \\ &\quad s\left[{e_2} + a{k_1}{\left| {{e_1}} \right|^{a - 1}}{e_2} + b{k_2}{\left| {{e_2}} \right|^{b - 1}}\left({b_0}{i_{{\mathrm{sw}}}} - M{\mathrm{sign}}(s) + d - \frac{1}{{b{k_2}}}{\left| {{e_2}} \right|^{2 - b}}(1 + a{k_1}{\left| {{e_1}} \right|^{a - 1}})\times {\mathrm{sign}}({e_2})\right)\right] = \\ &\quad s[b{k_2}{\left| {{e_2}} \right|^{b - 1}}(d - M{\mathrm{sign}}(s) + {b_0}{i_{{\mathrm{sw}}}})] = b{k_2}{\left| {{e_2}} \right|^{b - 1}}\left(ds - M\left| s \right| - {k_3}{\left| s \right|^{g + 1}} - {k_4}\int {\left| s \right|{\mathrm{d}}t} \right) . \end{split}$

(16)

当e₂≠0时，已知k₂、k₃、k₄、b均大于0，且$ \left| d \right| $≤M，那么此时有

$\dot V(s) \lt b{k_2}{\left| {{e_2}} \right|^{b - 1}}( - {k_3}{\left| s \right|^{g + 1}} - {k_4}\int {\left| s \right|{\mathrm{d}}t} ) \lt 0.$

(17)

此时，滑模函数s将在有限时间内到达滑模面s=0.

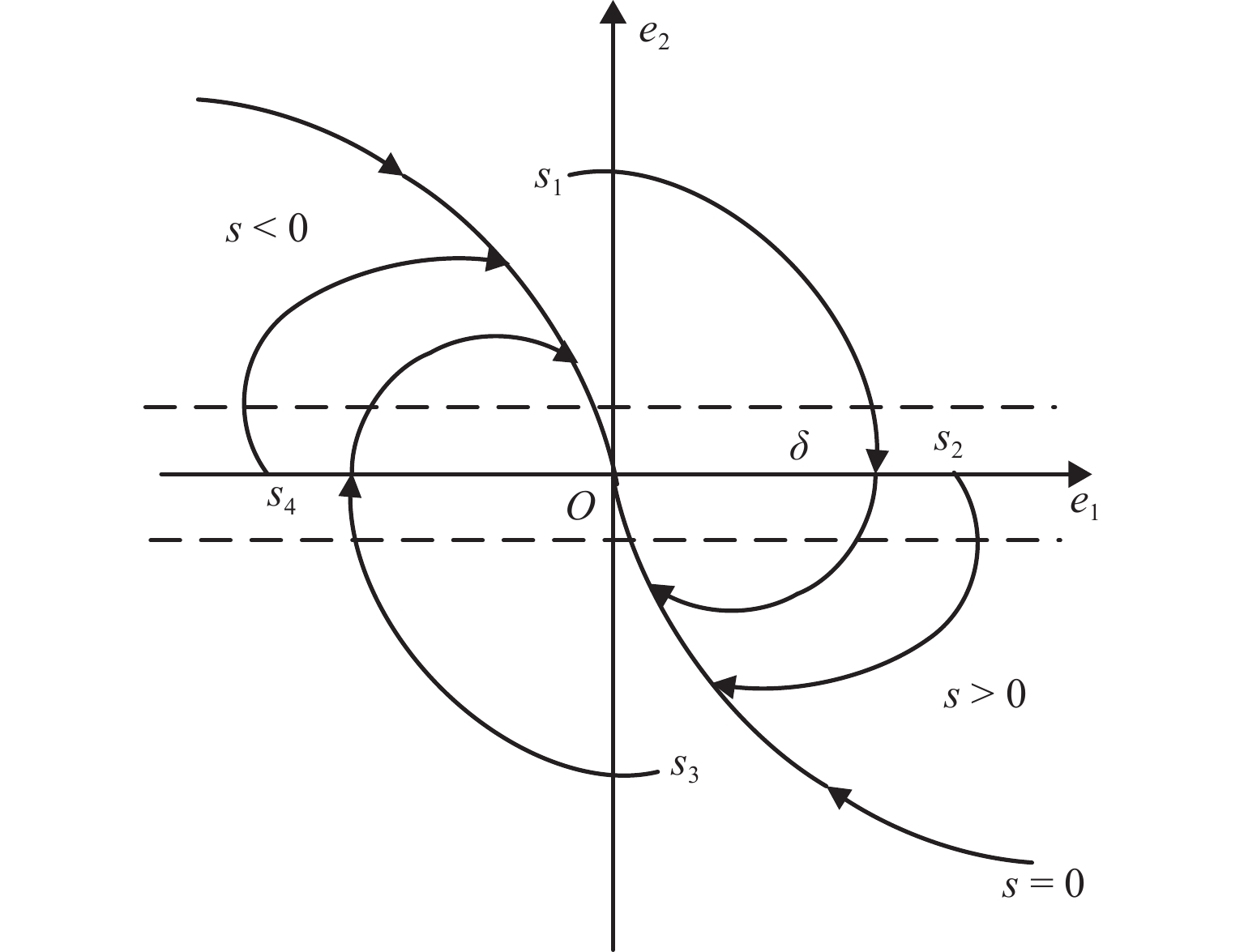
当e₂=0时，联立式（14）与式（5）中，有

${\dot e_2} = - {k_3}{\left| s \right|^g}{\mathrm{sign}}(s) - {k_4}\int {{\mathrm{sign}}(s){\mathrm{d}}t + d} - M{\mathrm{sign}}(s).$

(18)

根据式（18），当滑模函数s>0时，$ {\dot e_2} \lt 0 $；当滑模函数s<0时，$ {\dot e_2} \lt 0 $. 图5为该控制器下的系统相轨迹，以滑模函数s₂为例，e₂=0、$ {\dot e_2} \lt 0 $时，e₂必然会在某个邻域（0, + δ）内减小，此时滑模函数s₂会必然会向下运动；当滑模函数s₂到达邻域（0, + δ）时，e₂≠0，滑模函数s₂将会根据式（17）得出的结论到达滑模面s=0，滑模函数s₄同理.

图 5 AMBs系统相轨迹

Figure 5. Phase trajectory of AMB system

下载: 全尺寸图片幻灯片

3. 考虑NESO的SNFTSMC设计

3.1 ESO设计

集总干扰d是未知的，很难确定其具体的上界，而为了保证系统的稳定，一般上界M取值较大，将会加剧系统抖振. 为避免这种情况，本文通过设计ESO，并利用ESO对集总干扰d进行观测，得到较精确的观测值，然后将观测值补偿到控制器中. 本节先后分别对LESO与NESO进行研究与分析.

对于二阶的AMBs转子系统，将集总干扰d扩张为新的状态变量y₃，式（5）可以改写为

$\left\{ {\begin{array}{*{20}{l}} {{{\dot y}_1} = {y_2}}, \\ {{{\dot y}_2} = {b_0}i + {a_0}{y_1} + {y_3}}, \\ {{{\dot y}_3} = h}, \end{array}} \right.$

(19)

式中：h为集总干扰d的变化率.

根据式（19）可以写出LESO表达式为

$\left\{ {\begin{array}{*{20}{l}} {{{\dot {\textit{z}}}_1} = {{\textit{z}}_2} - {L_1}{\theta _1}}, \\ {{{\dot {\textit{z}}}_2} = {b_0}i + {a_0}{{\textit{z}}_1} + {{\textit{z}}_3} - {L_2}{\theta _1}}, \\ {{{\dot {\textit{z}}}_3} = - {L_3}{\theta _1}} , \end{array}} \right.$

(20)

式中：L₁、L₂、L₃为LESO增益，z₁、z₂、z₃分别为y₁、y₂、y₃的观测值，θ₁=z₁−y₁为观测误差.

式（20）减去式（19）得到误差状态方程为

$\left\{ {\begin{array}{*{20}{l}} {{{\dot \theta }_1} = {\theta _2} - {L_1}{\theta _1}}, \\ {{{\dot \theta }_2} = {a_0}{\theta _1} + {\theta _3} - {L_2}{\theta _1}}, \\ {{{\dot \theta }_3} = - h - {L_3}{\theta _1}} , \end{array}} \right.$

(21)

式中：θ₂=z₂−y₂，θ₃=z₃−y₃.

式（21）经过拉普拉斯变换后，有

$\left\{ {\begin{array}{*{20}{l}} {{\theta _2}(s) = (s + {L_1}){\theta _1}(s)}, \\ {{\theta _3}(s) = s{\theta _2}(s) + ({L_2} - {a_0}){\theta _1}(s)}, \\ {h(s) = s{y_3}(s) = - s{\theta _3}(s) - {L_3}{\theta _1}(s)} . \end{array}} \right.$

(22)

整理式（22），得到θ₃与−y₃之间的传递函数为

$\frac{{{\theta _3}}}{{ - {y_3}}} = \frac{{{s^3} + {L_1}{s^2} + ({L_2} - {a_0})s}}{{{s^3} + {L_1}{s^2} + ({L_2} - {a_0})s + {L_3}}}.$

(23)

为了使系统能够稳定，假设式（23）有3个极点(s₁、s₂、s₃)都位于左半平面，p₁=−ω₀、p₂=−0.5ω₀ + j0.5ω₀、p₃=−0.5ω₀−j0.5ω₀，ω₀为带宽并大于0，那么有

${s^3} + {L_1}{s^2} + ({L_2} - {a_0})s + {L_3} = (s - {p_1})(s - {p_2})(s - {p_3}).$

(24)

可以解得

$\left[ {\begin{array}{*{20}{c}} {{L_1}}&{{L_2}}&{{L_3}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {2{\omega _0}}&{1.5\omega _0^2 + {a_0}}&{0.5\omega _0^3} \end{array}} \right].$

(25)

将式（25）带到式（23）中可得

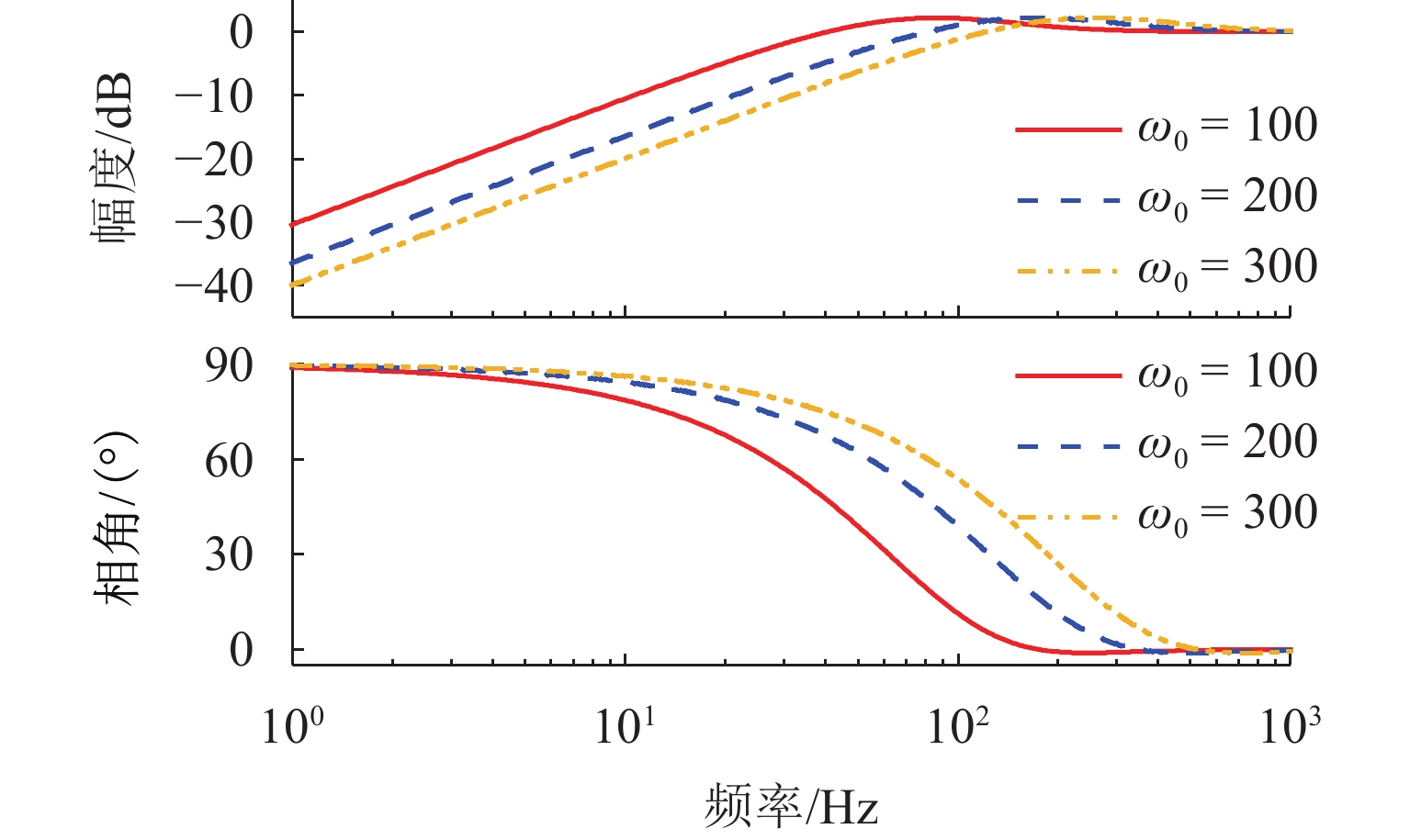
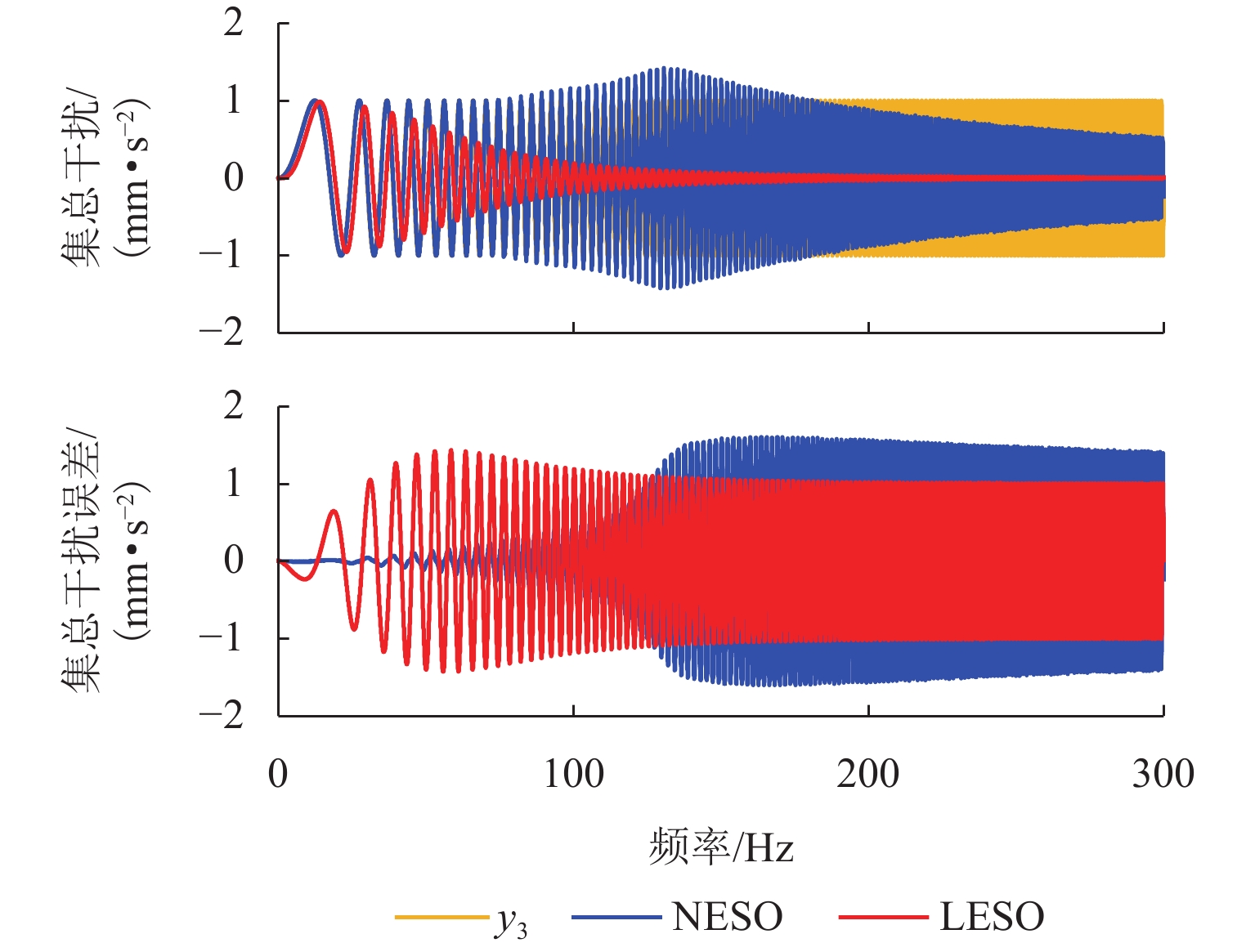
$\frac{{{\theta _3}}}{{ - {y_3}}} = \frac{{{s^3} + 2{\omega _0}{s^2} + 1.5\omega _0^2s}}{{{s^3} + 2{\omega _0}{s^2} + 1.5\omega _0^2s + 0.5\omega _0^3}}.$

(26)

根据式（26）做出不同带宽下的Bode图，如图6. 图6中：y₃频率较低时，z₃对y₃的跟踪效果较好；随着y₃频率的增大，z₃对y₃的跟踪性能逐渐变差；带宽增大后，z₃对y₃跟踪效果逐渐变好，但带宽太大容易对系统中其他噪声敏感.

图 6 不同带宽下的Bode图

Figure 6. Bode plots with different bandwidths

下载: 全尺寸图片幻灯片

由于LESO对集总扰动观测精度有限，现采用NESO对集总干扰进行观测，将式（20）改写为

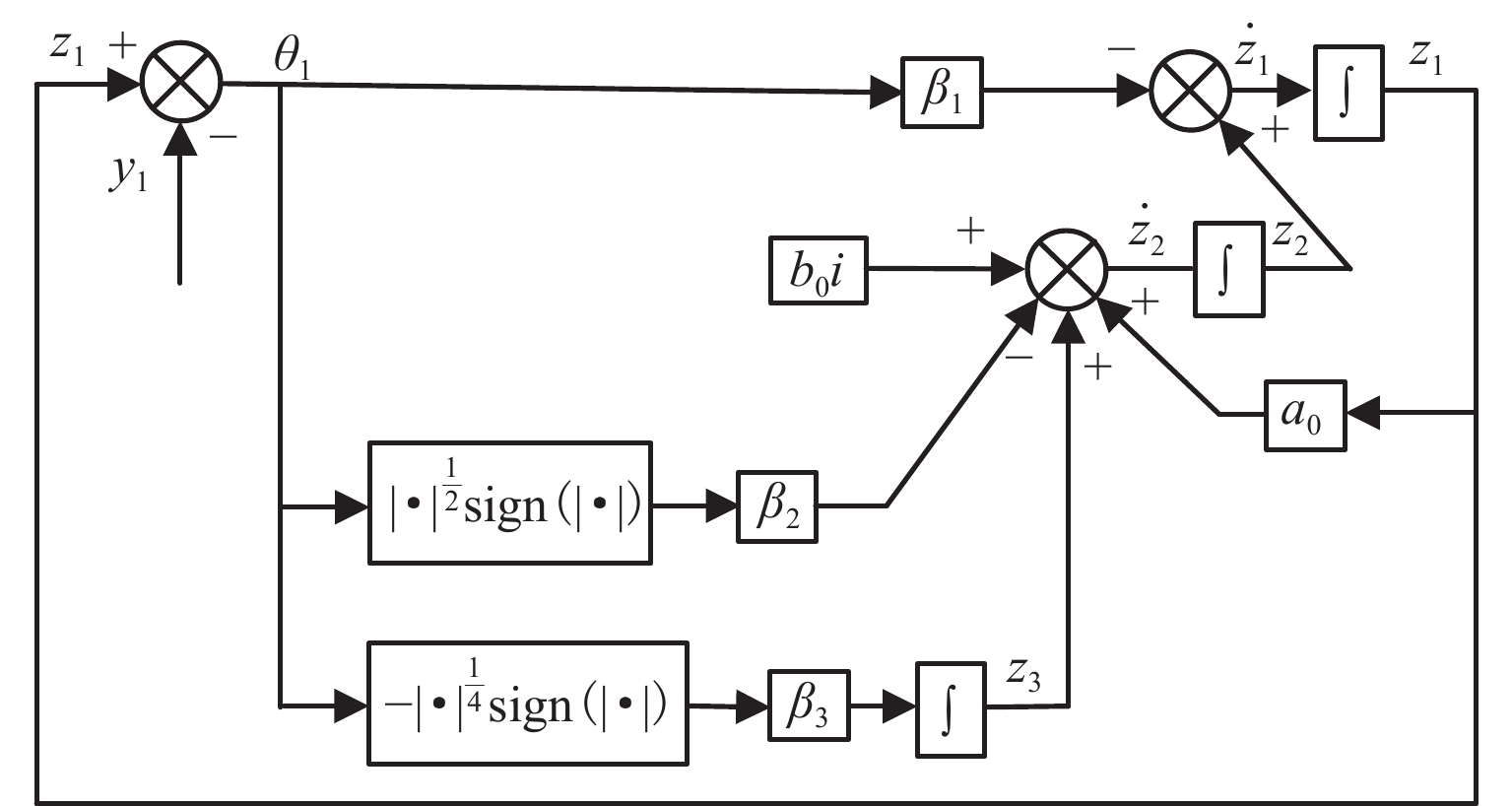
$\left\{ {\begin{array}{*{20}{l}} {{{\dot {\textit{z}}}_1} = {{\textit{z}}_2} - {\beta _1}{u_1}({\theta _1})}, \\ {{{\dot {\textit{z}}}_2} = {b_0}i + {a_0}{{\textit{z}}_1} + {{\textit{z}}_3} - {\beta _2}{u_2}({\theta _1})}, \\ {{{\dot {\textit{z}}}_3} = - {\beta _3}{u_3}({\theta _1})} , \end{array}} \right.$

(27)

式中：β₁、β₂、β₃为待设计的观测器增益，均大于0；u₁(θ₁)、u₂(θ₁)、u₃(θ₁)为关于θ₁的非线性函数，如式（28）.

$\left\{ {\begin{array}{*{20}{l}} {{u_1}({\theta _1}) = {\theta _1}}, \\ {{u_2}({\theta _1}) = {{\left| {{\theta _1}} \right|}^{\frac{1}{2}}}{\mathrm{sign}}({\theta _1})}, \\ {{u_3}({\theta _1}) = {{\left| {{\theta _1}} \right|}^{\frac{1}{4}}}{\mathrm{sign}}({\theta _1})}. \end{array}} \right.$

(28)

NESO的参数一般很难通过理论去整定，通常根据经验来进行设计. 选择合适的β₁、β₂、β₃，能够使得观测误差θ₁、θ₂、θ₃在有限时间内收敛到0.

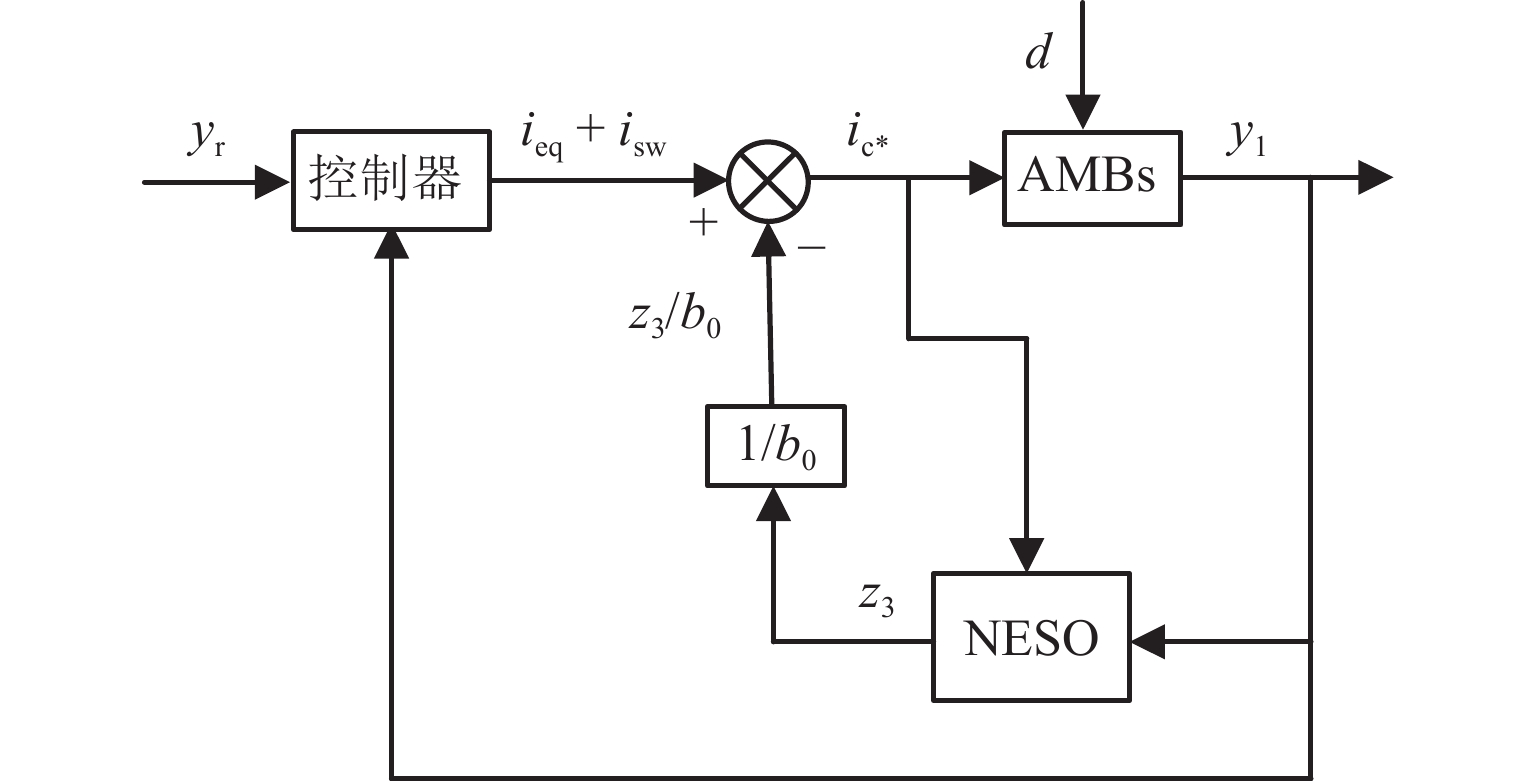
NESO的结构框图如7.

图 7 NESO结构图

Figure 7. Structure of NESO

下载: 全尺寸图片幻灯片

3.2 含NESO的SNFTSMC稳定性分析

式（14）中所提到的sign函数切换增益为$ - ({k_3}{\left| s \right|^g} + M)/{b_0} $，其中$ - {k_3}{\left| s \right|^g}/{b_0} $的大小与系统状态有关，产生的抖振很小，而$ - M/{b_0} $为常值，会导致系统产生较大抖振. 因此，需要通过NESO对系统抖振进行补偿，进一步将控制器设计为

${i_{{\mathrm{c}} * }} = {i_{{\mathrm{eq}}}} + {i_{{\mathrm{sw}}}} - \frac{1}{{{b_0}}}{{\textit{z}}_3}.$

(29)

接着，为了验证所提方法对系统稳定性产生的影响，对式（29）进行李亚普诺夫稳定性分析，即

$\begin{split} & \dot V(s) = s\dot s = s[b{k_2}{\left| {{e_2}} \right|^{b - 1}}(d + {b_0}{i_{{\mathrm{sw}}}} - {{\textit{z}}_3})]= \\ &\quad b{k_2}{\left| {{e_2}} \right|^{b - 1}}\left(ds - {{\textit{z}}_3}s - {k_3}{\left| s \right|^{g + 1}} - {k_4}\int {\left| s \right|{\mathrm{d}}t} \right) \lt \\ &\quad b{k_2}{\left| {{e_2}} \right|^{b - 1}}\left( - {k_3}{\left| s \right|^{g + 1}} + \left| {{\theta _3}} \right|\left| s \right| - {k_4}\int {\left| s \right|{\mathrm{d}}t} \right). \end{split}$

(30)

根据对NESO的设计，θ₃会收敛到0，可得到：

$\dot V(s) \lt b{k_2}{\left| {{e_2}} \right|^{b - 1}}\left( - {k_3}{\left| s \right|^{c + 1}} - {k_4}\int {\left| s \right|{\mathrm{d}}t} \right) \lt 0.$

(31)

通过分析得知，滑模函数s能够在有限时间内到达滑模面s=0，有效证明了所提方法的稳定性，进而搭建如图8所示的AMBs系统整体控制结构.

图 8 AMBs系统整体控制结构

Figure 8. Overall control structure of AMB system

下载: 全尺寸图片幻灯片

4. 仿真分析

表1为AMBs具体参数.

表 1 AMBs参数

Table 1. Parameters of AMBs

参数	值
磁极面积/mm²	720
匝数/圈	150
气隙长度/mm	0.4
偏置电流/A	2
转子质量/kg	15
电流刚度系数/（N·A⁻¹）	939.5
位移刚度系数/（N·mm⁻¹）	4697.5

下载: 导出CSV

| 显示表格

为了能够对比出SNFTSMC的优越性能，在验证中加入传统SMC，表2为各个控制器参数.

表 2 控制器参数

Table 2. Parameters of controller

控制器	值
SNFTSMC	k₁=1、k₂=0.1、k₃=80、k₄=50、a=2.5、b=1.5、γ=1.5、λ=1、η=0.5、M=15
SMC	k₁=30、k₂=50、c=10、M=15

下载: 导出CSV

| 显示表格

忽略集总扰动时SNFTSMC、SMC分别为

$\left\{ {\begin{array}{*{20}{l}} {{i_{{\mathrm{SNFTSMC}}}} = {i_{{\mathrm{eq}}}} + {i_{{\mathrm{sw}}}}}, \\ {{i_{{\mathrm{SMC}}}}{\text{ }} = \dfrac{1}{{{b_0}}}[{{\ddot y}_{\mathrm{r}}} - c{e_2} - {a_0}{y_1} - {k_1}s - {k_2}{\mathrm{sign}}(s)]} . \end{array}} \right.$

(32)

定义控制电流平均值为

${i_{{\mathrm{avg}}}} = \sum\nolimits_{j = 1}^N {\frac{{\left| {{i_{j}}} \right|}}{N}},$

(33)

式中：i_j为第j个采样点的电流值，1≤j≤N ，N为采样点数.

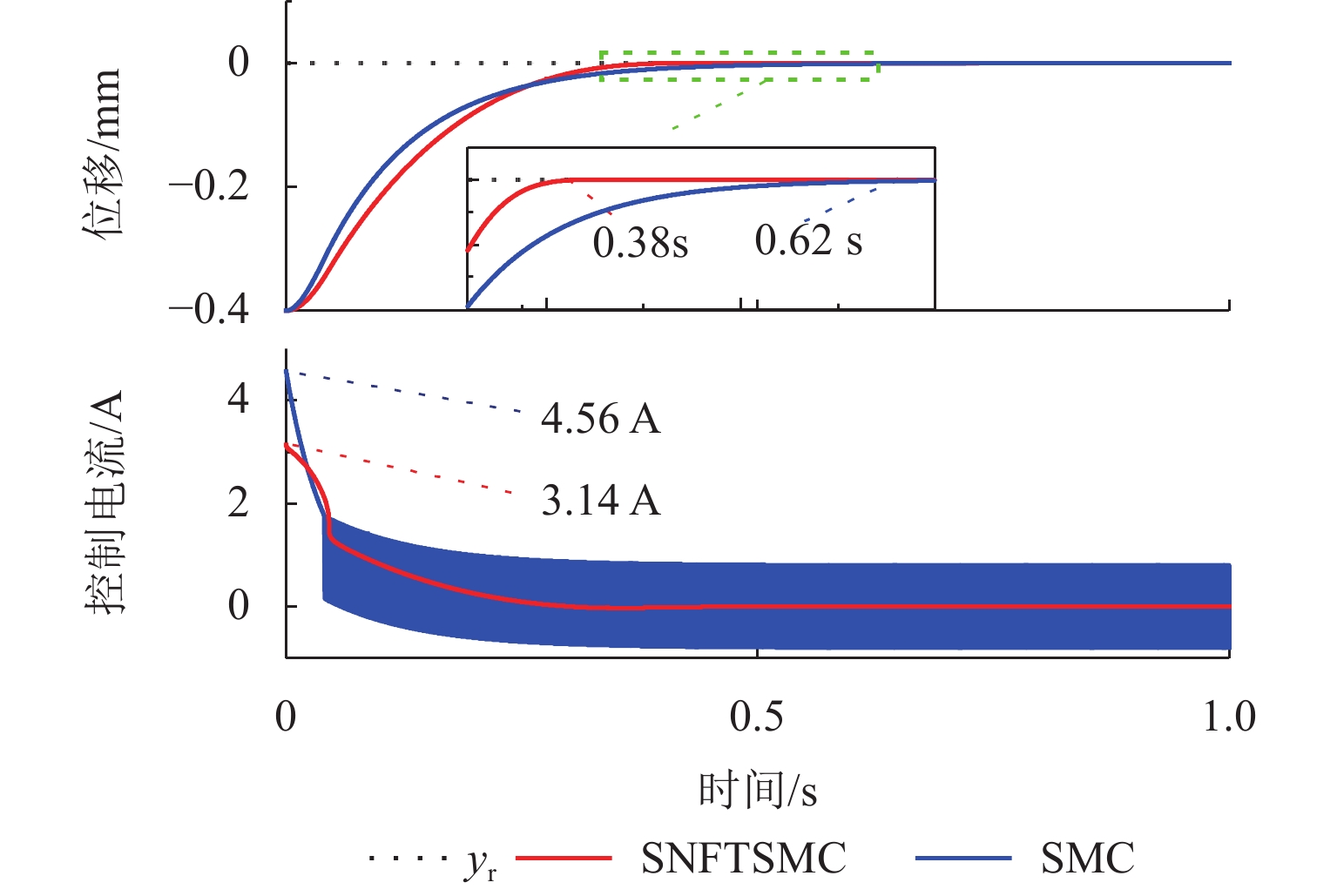
根据式（32）中的控制器进行仿真，图9为起浮测试下的位移与控制电流. SNFTSMC、SMC到达目标位置的时间分别为0.38、0.62 s，SNFTSMC、SMC的最大控制电流分别为3.14、4.56 A. 根据式（33）得到SNFTSMC与SMC的电流平均值为分别为0.24、0.89 A.

图 9 转子起浮位移和电流信号

Figure 9. Displacement and current signal in case of rotor suspension

下载: 全尺寸图片幻灯片

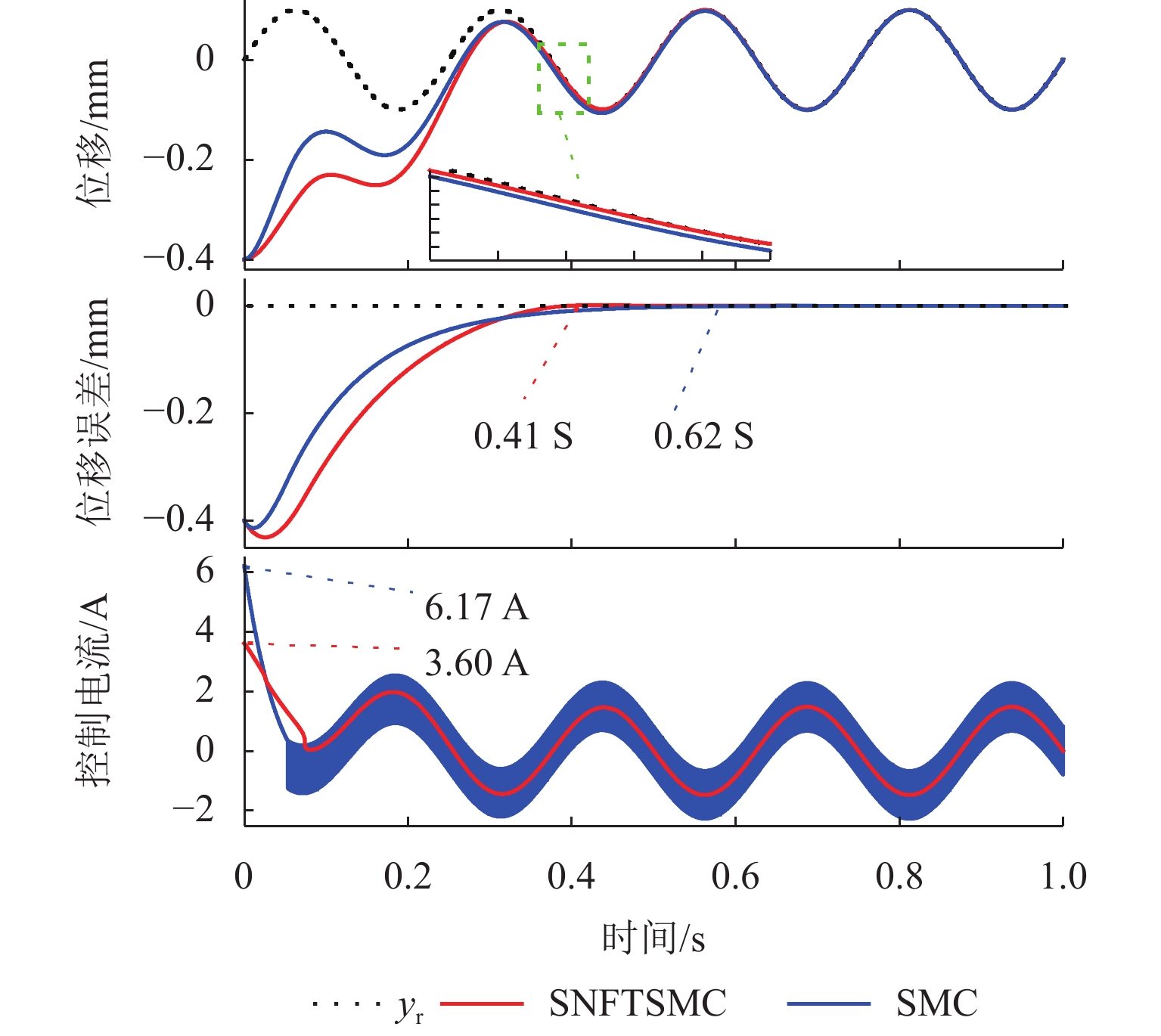
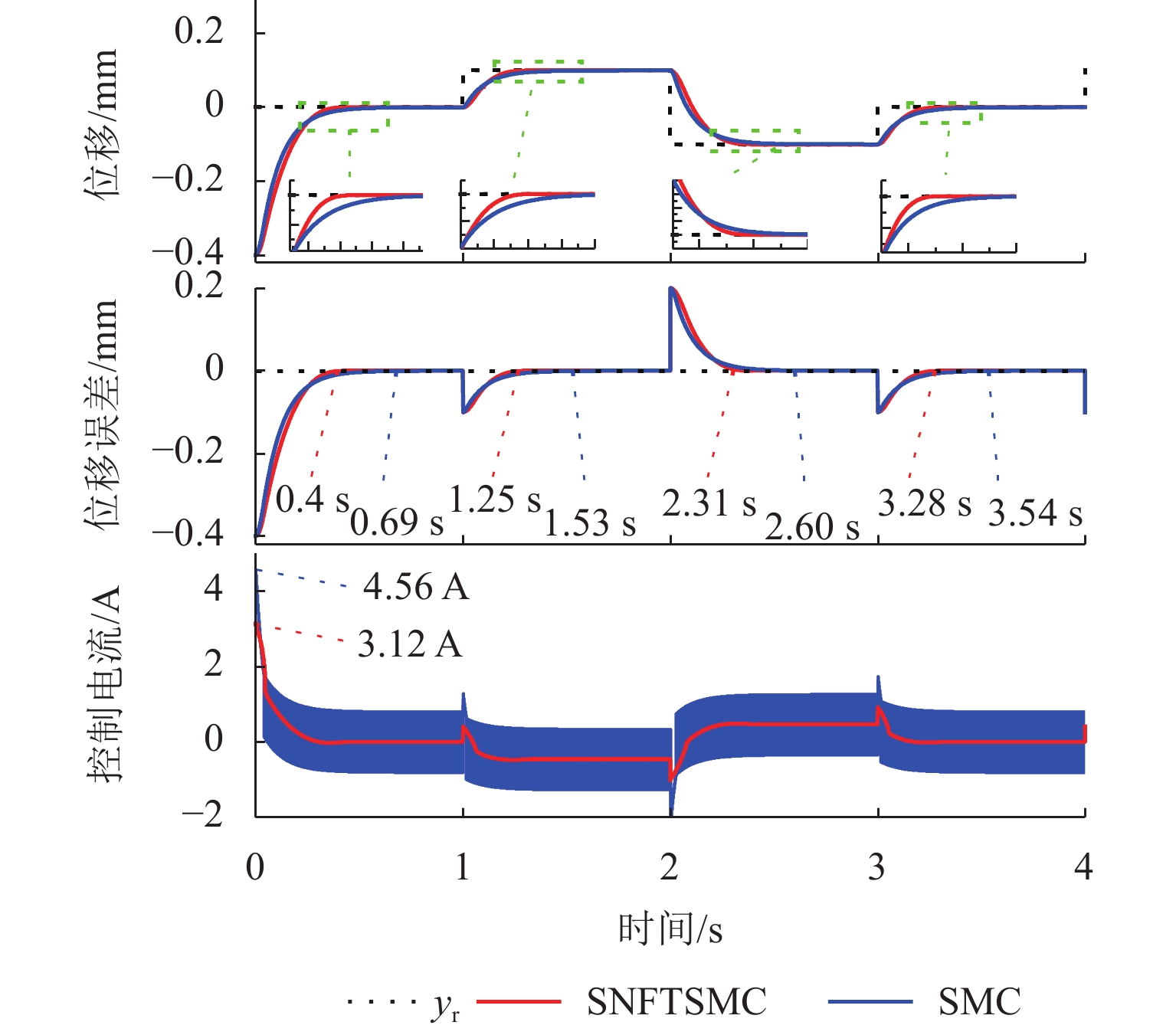
为探究控制器的追踪性能，对正弦波、方波进行追踪. 图10为正弦追踪下的结果，SNFTSMC、SMC追踪到正弦波的时间分别为0.41、0.62 s，控制电流最大值分别为3.60、6.17 A，电流平均值分别为1.04、1.16 A. 图11为方波追踪下的仿真结果，将方波追踪中4个阶段的稳定时间间隔累加起来，SNFTSMC、SMC所用时间分别为1.24、2.36 s；控制电流最大值分别为3.12、4.56 A；电流平均值分别为0.24、0.89 A.

图 10 正弦追踪中转子位移和电流信号

Figure 10. Displacement and current signal of rotor under sinusoidal trace

下载: 全尺寸图片幻灯片

图 11 方波追踪中转子位移和电流信号

Figure 11. Displacement and current signal of rotor under square wave trace

下载: 全尺寸图片幻灯片

为对比LESO与NESO的观测性能，对正弦扰动sin（2πf_ot）进行观测，其频率f_o从0增加到300 Hz. LESO带宽为500，NESO的观测增益β₁、β₂、β₃分别为15000、3000、50000. 图12为2种ESO对正弦信号的观测结果. 随着频率增大，2种ESO的观测性能均随之下降；在低频段中NESO观测器性能较好，其观测误差很小，而LESO在低频段中其观测误差依然很大，并且此时还是在LESO带宽取值较大的情况下. 因此，LESO观测性能不如NESO. 所以选用NESO对集总干扰进行观测.

图 12 ESO观测结果

Figure 12. Observation results with ESO

下载: 全尺寸图片幻灯片

将外部扰动与未建模部分对考虑到系统中，SNFTSMC的控制器设计为式（14），SMC设计为

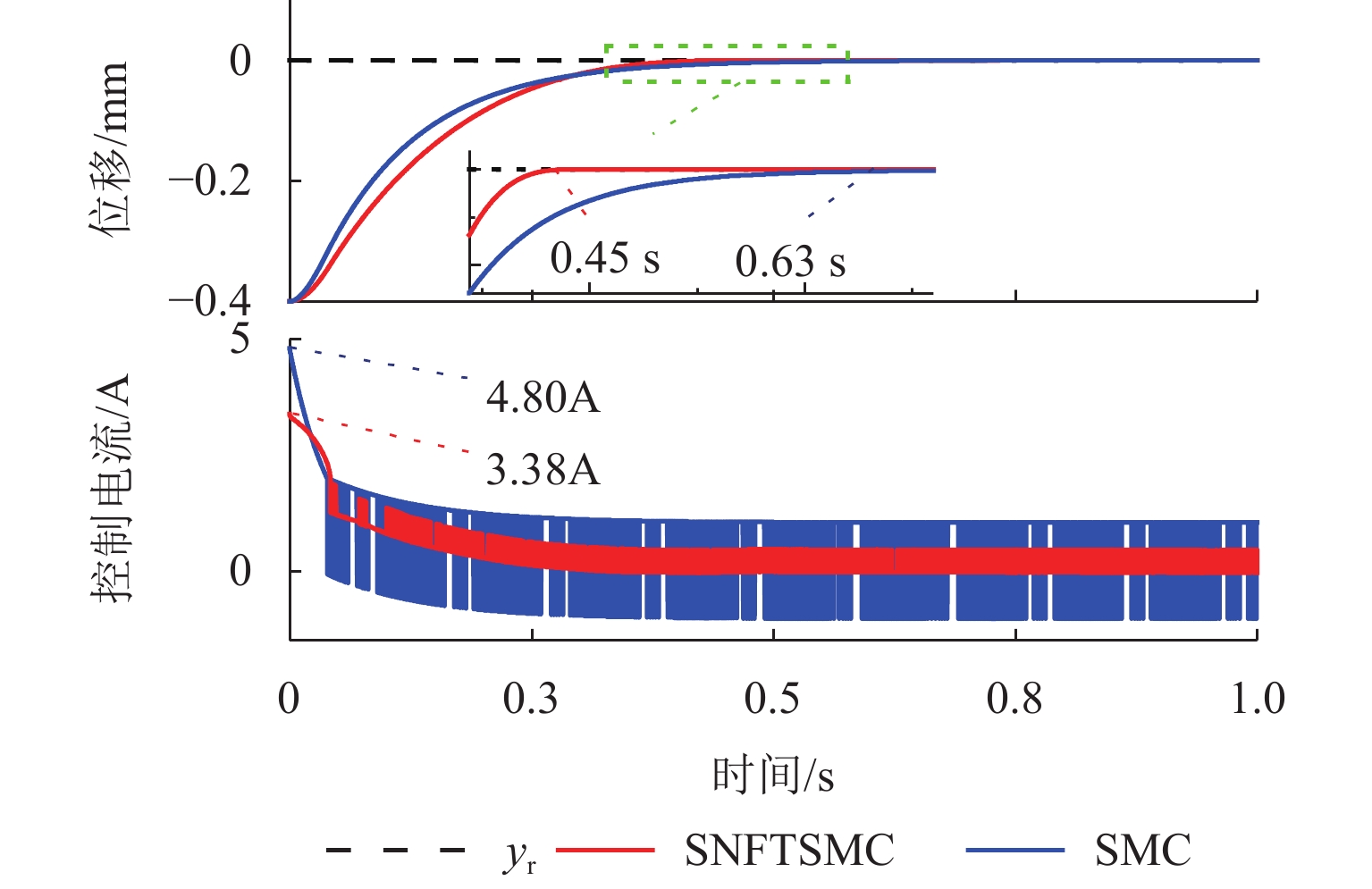
${i_{{\mathrm{SMC}}}} = \frac{1}{{{b_0}}}[{\ddot y_r} - c{e_2} - {a_0}{y_1} - {k_1}s - ({k_2} + M){\mathrm{sign}}(s)].$

(34)

假设集总干扰d=−12.5 + 2.5sin（20πt），采用考虑到集总干扰而设计的控制器进行仿真，图13为抗干扰测试下的转子位移波形与控制电流波形. 由图13可知：SNFTSMC、SMC到达目标位置的时间分别为0.45、0.63 s，相较于没有集总干扰的情况下系统收敛时间增加；控制电流最大值分别为3.38、4.80 A，控制电流平均值分别为0.43、1.18 A；考虑到扰动后控制电流最大值与控制电流平均值都略微增大，并且SMC抖振加剧、SNFTSMC产生了小幅度抖振.

图 13 集总干扰作用下转子起浮位移和电流信号

Figure 13. Displacement and current signal in case of rotor suspension under lumped interference

下载: 全尺寸图片幻灯片

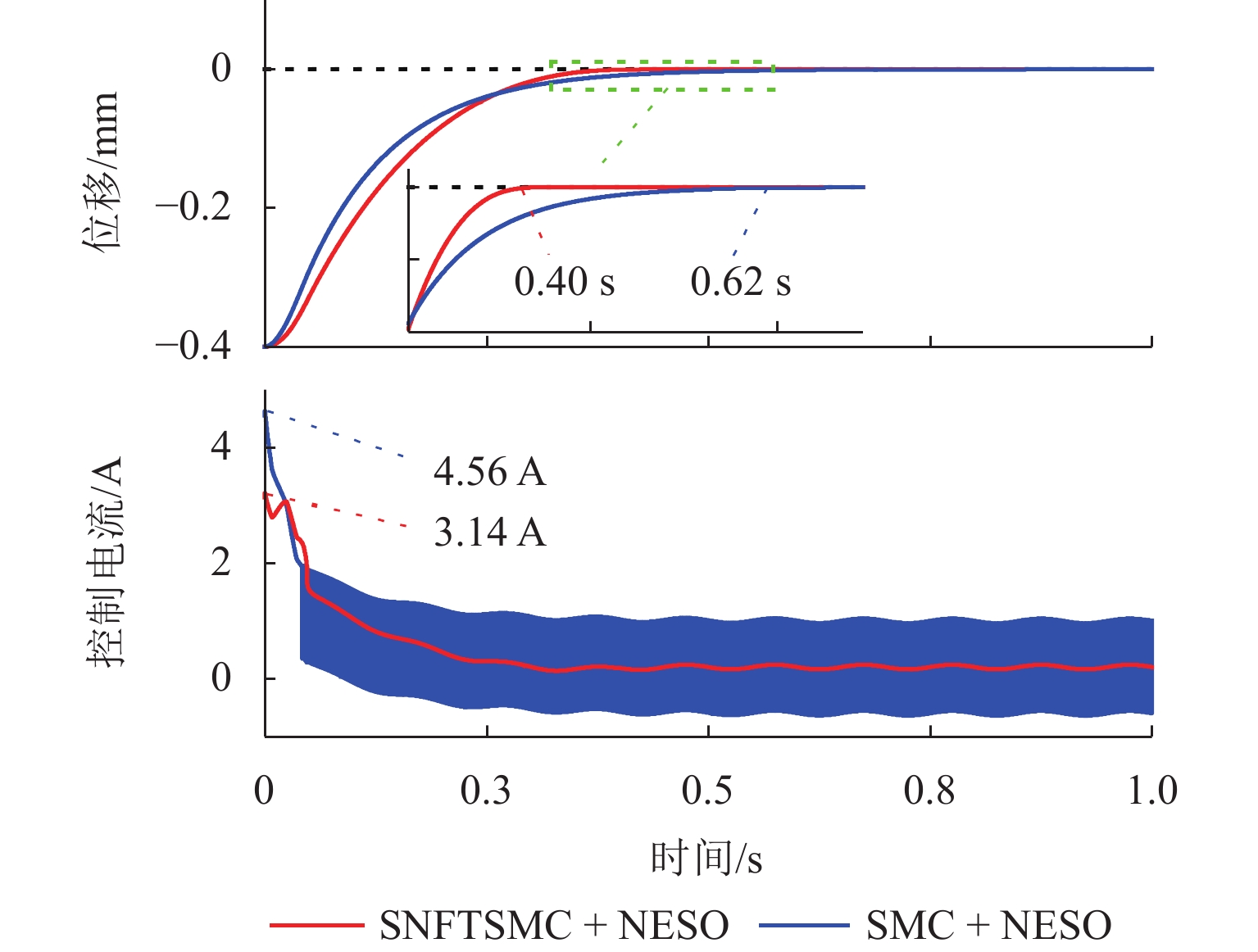
集总干扰的存在会增大抖振，将SNFTSMC与NESO相结合，以此来减小由集总扰动引起的系统抖振. 图14为SNFTSMC+NESO、SMC+NESO这2种控制方法的仿真结果. 由图14可知：SNFTSMC+NESO、SMC+NESO到达目标位置的时间分别为0.40 s、0.62 s，与没有集总干扰的情况下系统收敛时间相近，即NESO能够消除集总干扰带来的影响；SNFTSMC+NESO、SMC+NESO在稳定时位移误差均为0，控制电流最大值分别为3.14、4.56 A，控制电流平均值分别为0.44、0.90 A.

图 14 NESO补偿后转子起浮位移和电流信号

Figure 14. Displacement and current signal in case of rotor suspension with NESO compensation

下载: 全尺寸图片幻灯片

5. 实验验证

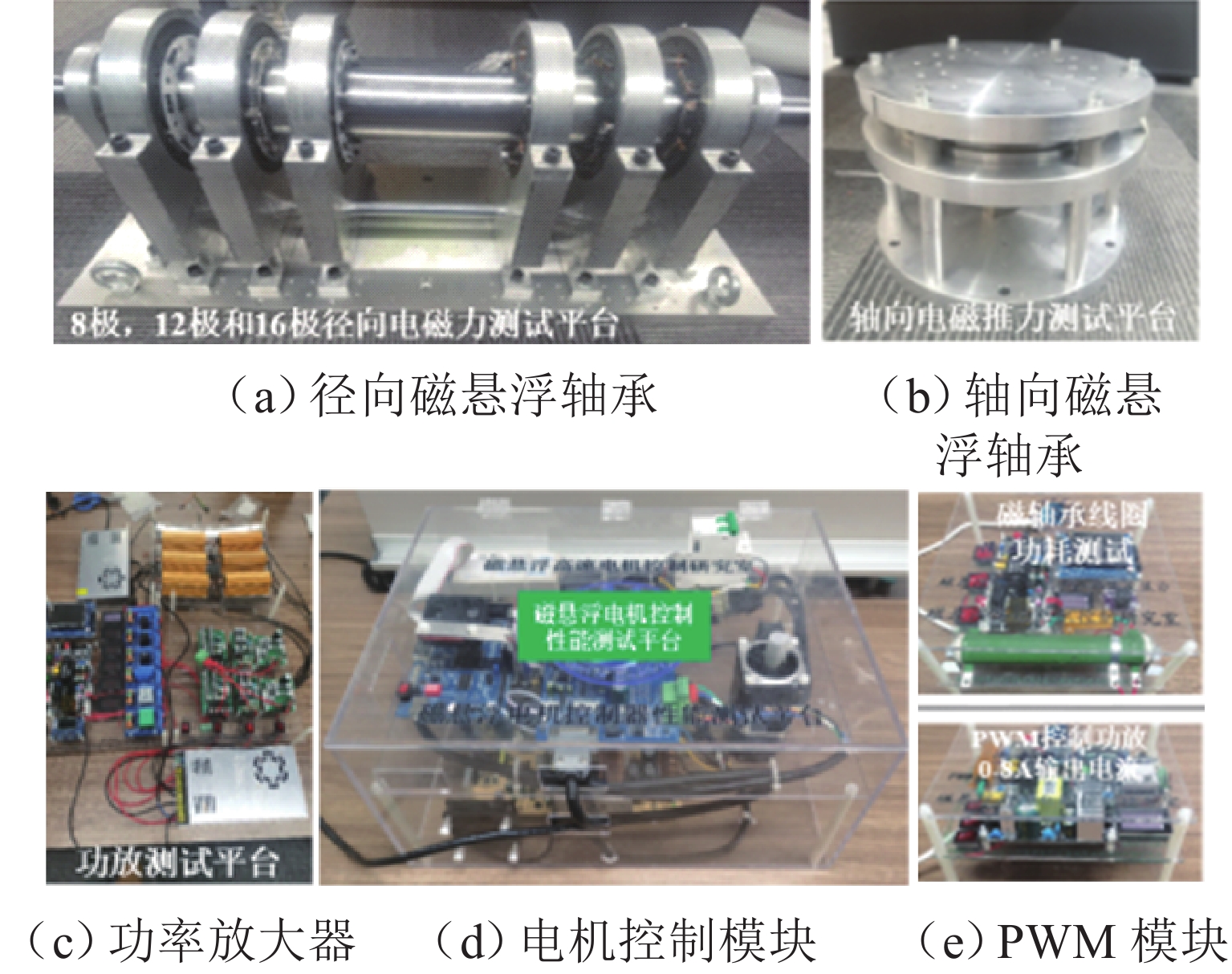
为验证所提方法的正确性和有效性，搭建了基于RT-Lab的磁悬浮轴承转子系统实验平台. 实验装置由磁悬浮电机性能测试平台、功放测试平台、径向磁悬浮轴承和轴向磁悬浮轴承等组成，如图15所示.

图 15 磁悬浮高速电机转子系统实验平台

Figure 15. Experimental platform of rotor system with magnetic suspension high-speed motor

下载: 全尺寸图片幻灯片

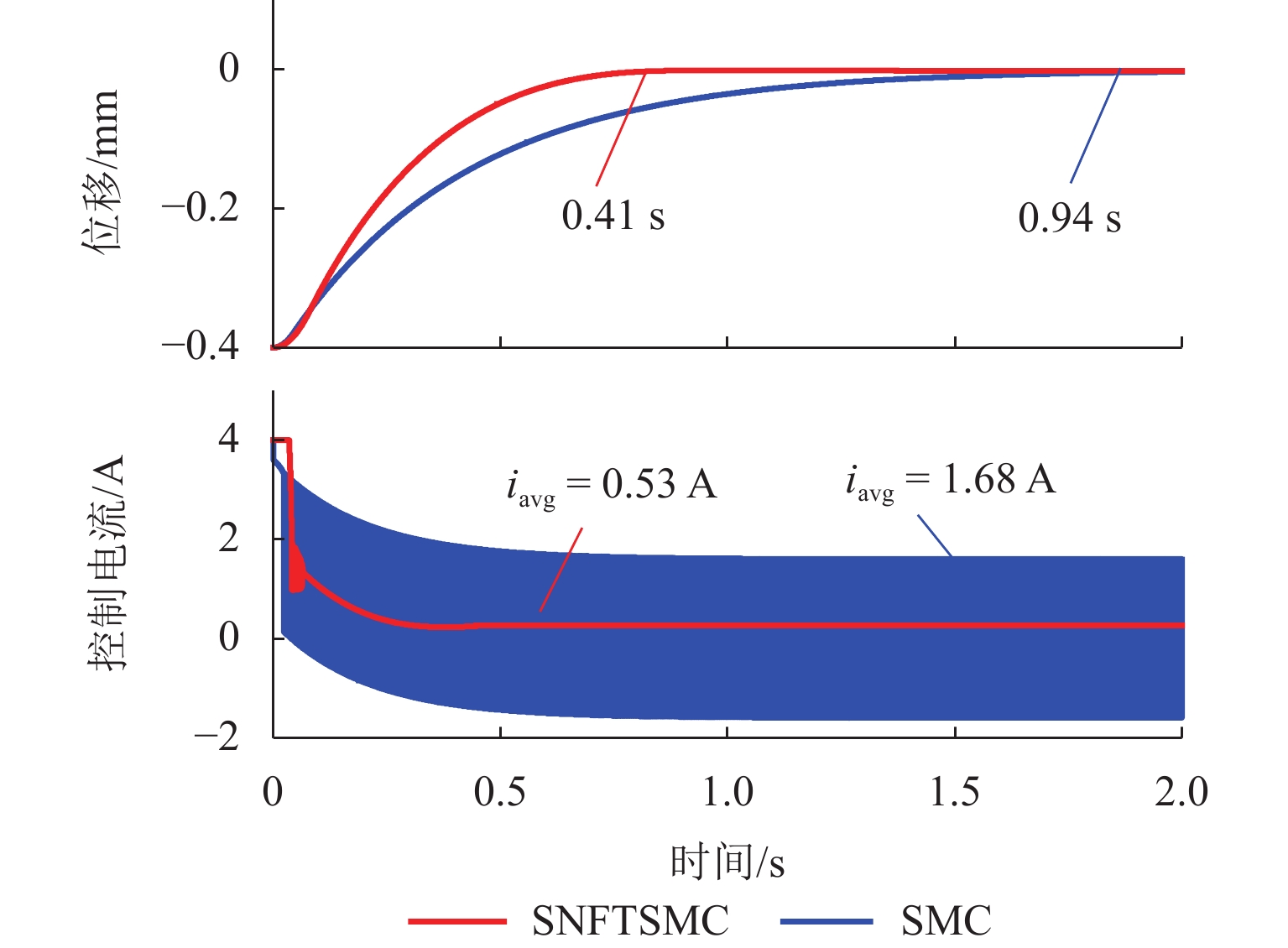
首先，通过采用SNFTSMC、SMC 2种控制器进行转子起伏测试，转子起浮位移和电流信号如图16所示. 可以得知，采用SNFTSMC、SMC转子从底端上升到目标位置所用的时间分别为0.41、0.94 s，并且SMC的控制电流存在剧烈的抖振，SNFTSMC、SMC的电流平均值分别为0.53、1.68 A.

图 16 转子起浮位移和电流信号

Figure 16. Displacement and current signal in case of rotor suspension

下载: 全尺寸图片幻灯片

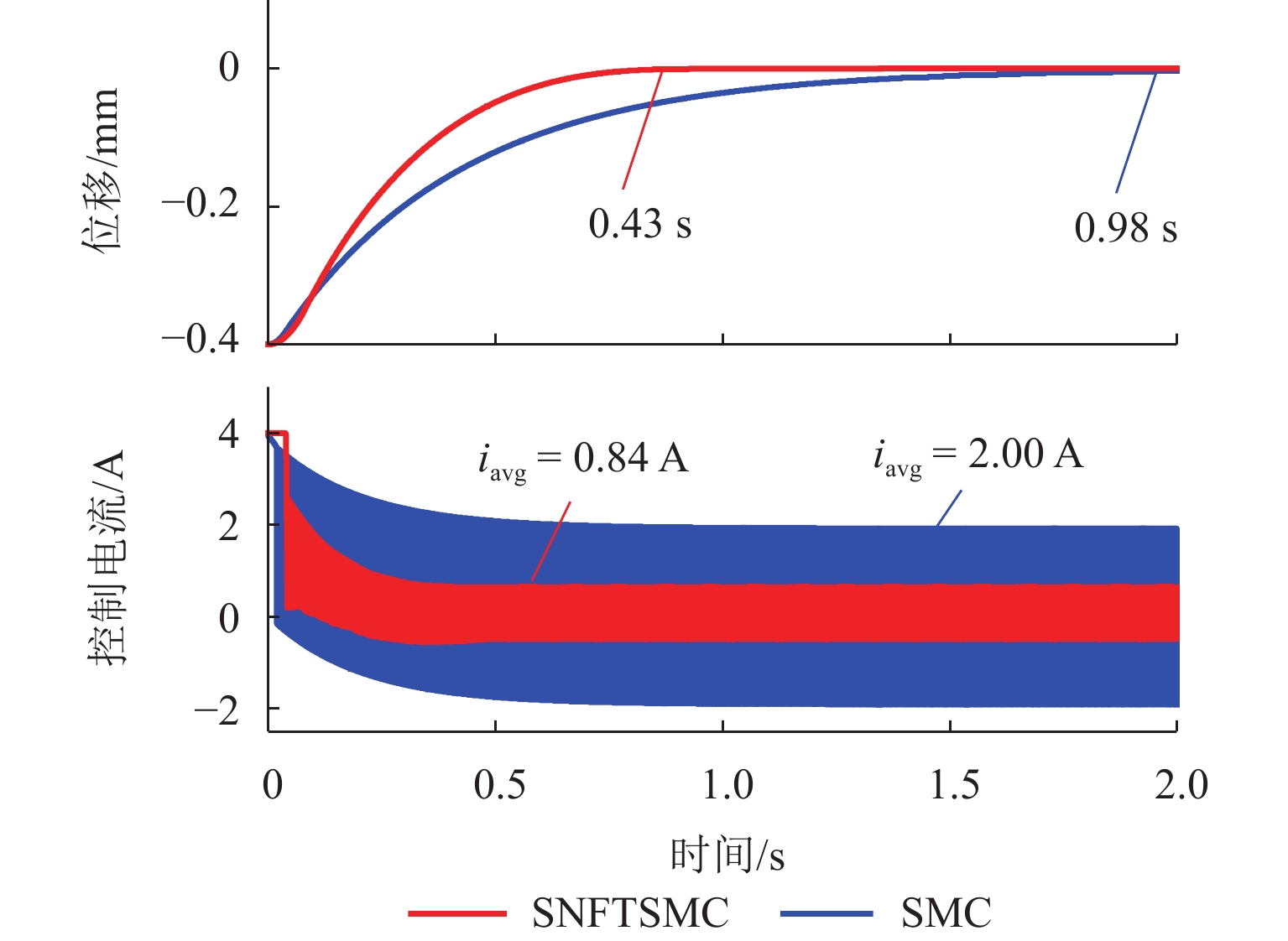
其次，为验证控制器的抗干扰性能，对转子施加正弦扰动进行起浮测试，转子起浮位移和电流信号如图17所示. 图17中SNFTSMC、SMC到达目标位置所用的时间分别为0.43、0.98 s，SNFTSMC、SMC的电流平均值分别为0.84、2.00 A，并且SNFTSMC也产生了抖振.

图 17 扰动作用下转子起浮位移和电流信号

Figure 17. Displacement and current signal in case of rotor suspension under interference

下载: 全尺寸图片幻灯片

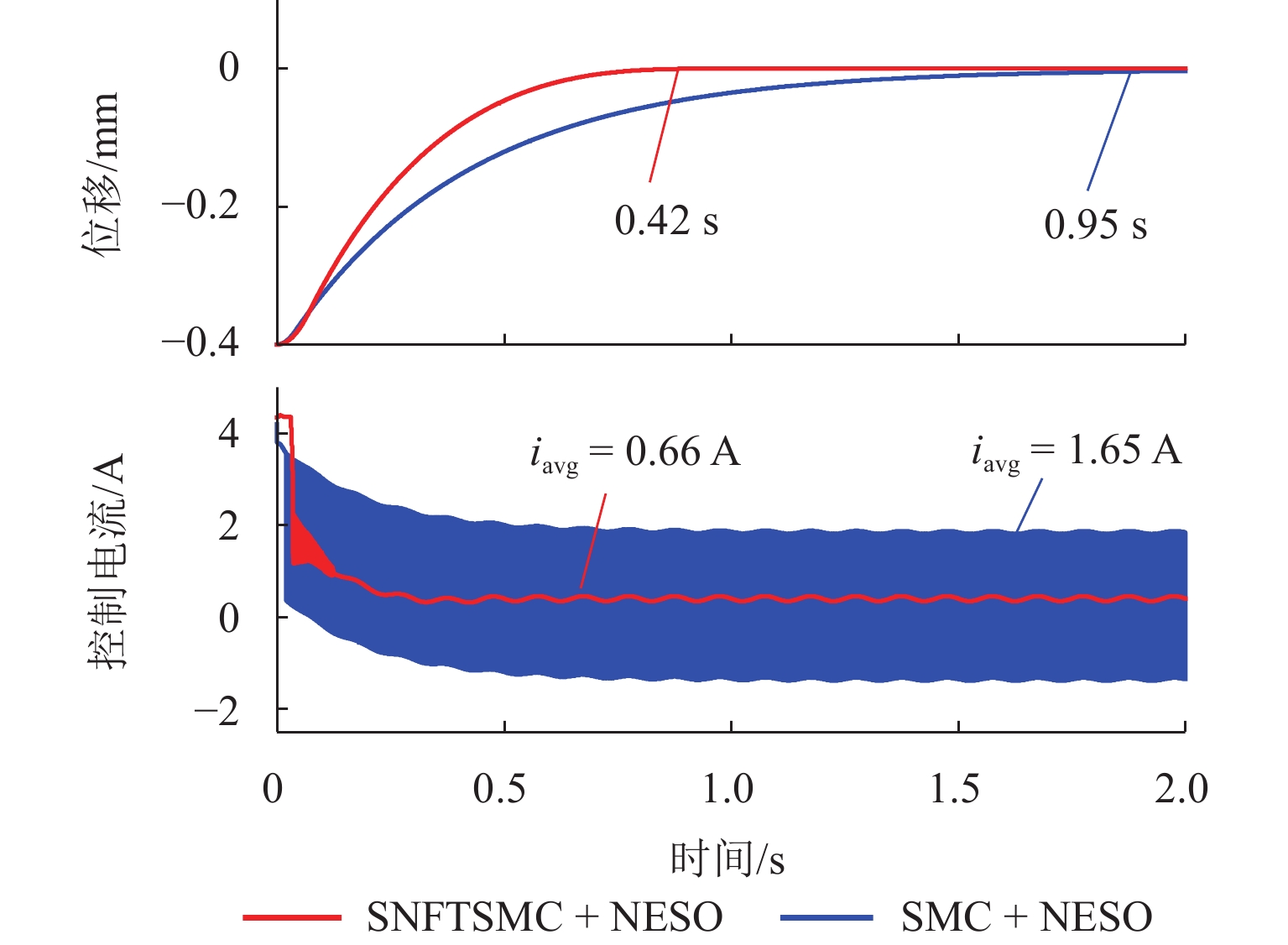
最后，引入NESO来对干扰进行补偿，转子位移和控制电流如图18所示. 图18中SNFTSMC+NESO和SMC+NESO到达目标位置所用的时间分别为0.42、0.95 s，其电流平均值分别为1.65、0.66 A，2种控制器下的电流抖振得到了有效减小.

图 18 NESO补偿后转子起浮位移和电流信号

Figure 18. Displacement and current signal in case of rotor suspension with NESO compensation

下载: 全尺寸图片幻灯片

6. 结　论

1）工程设计中由于考虑算法的简明性，通常会采用传统滑模控制器，若要提高主动磁悬浮轴承转子位置的动态控制性能，可将传统滑模控制率改进为本文所提出超螺旋趋近律.

2）非奇异快速终端滑模函数的设计能够使系统误差得到快速收敛，而超螺旋趋近律利用幂函数以及对滑模函数的积分使得切换增益在滑模面s=0处较小，实际工况中可根据快速性和鲁棒性要求进行选择滑模函数和趋近律的设计.

3）引入的NESO能够对系统内外扰动进行观测并补偿到系统中，有效减小扰动对控制结果的影响，但实际工况下观测值补偿可能是离线的，在线补偿对控制器设计要求较高. 特别是要考虑观测器引起的相位滞后，在控制器设计过程中选择合适的前馈补偿将相位进行补偿.

致谢：感谢陕西省教育厅一般专项（青年）23JK0339资助；海洋工程全国重点实验室（上海交通大学）专项经费号GKZD010089.

图 1 单自由度AMBs系统原理

Figure 1. Principle of single-degree-of-freedom AMB system

下载: 全尺寸图片幻灯片

图 2 差动控制原理图

Figure 2. Principle of differential control

下载: 全尺寸图片幻灯片

图 3 非奇异快速终端滑模函数结构

Figure 3. Structure of non-singular fast terminal sliding mode function

下载: 全尺寸图片幻灯片

图 4 超螺旋趋近律结构

Figure 4. Structure of super-twisting reaching law

下载: 全尺寸图片幻灯片

图 5 AMBs系统相轨迹

Figure 5. Phase trajectory of AMB system

下载: 全尺寸图片幻灯片

图 6 不同带宽下的Bode图

Figure 6. Bode plots with different bandwidths

下载: 全尺寸图片幻灯片

图 7 NESO结构图

Figure 7. Structure of NESO

下载: 全尺寸图片幻灯片

图 8 AMBs系统整体控制结构

Figure 8. Overall control structure of AMB system

下载: 全尺寸图片幻灯片

图 9 转子起浮位移和电流信号

Figure 9. Displacement and current signal in case of rotor suspension

下载: 全尺寸图片幻灯片

图 10 正弦追踪中转子位移和电流信号

Figure 10. Displacement and current signal of rotor under sinusoidal trace

下载: 全尺寸图片幻灯片

图 11 方波追踪中转子位移和电流信号

Figure 11. Displacement and current signal of rotor under square wave trace

下载: 全尺寸图片幻灯片

图 12 ESO观测结果

Figure 12. Observation results with ESO

下载: 全尺寸图片幻灯片

图 13 集总干扰作用下转子起浮位移和电流信号

Figure 13. Displacement and current signal in case of rotor suspension under lumped interference

下载: 全尺寸图片幻灯片

图 14 NESO补偿后转子起浮位移和电流信号

Figure 14. Displacement and current signal in case of rotor suspension with NESO compensation

下载: 全尺寸图片幻灯片

图 15 磁悬浮高速电机转子系统实验平台

Figure 15. Experimental platform of rotor system with magnetic suspension high-speed motor

下载: 全尺寸图片幻灯片

图 16 转子起浮位移和电流信号

Figure 16. Displacement and current signal in case of rotor suspension

下载: 全尺寸图片幻灯片

图 17 扰动作用下转子起浮位移和电流信号

Figure 17. Displacement and current signal in case of rotor suspension under interference

下载: 全尺寸图片幻灯片

图 18 NESO补偿后转子起浮位移和电流信号

Figure 18. Displacement and current signal in case of rotor suspension with NESO compensation

下载: 全尺寸图片幻灯片

表 1 AMBs参数

Table 1. Parameters of AMBs

参数	值
磁极面积/mm²	720
匝数/圈	150
气隙长度/mm	0.4
偏置电流/A	2
转子质量/kg	15
电流刚度系数/（N·A⁻¹）	939.5
位移刚度系数/（N·mm⁻¹）	4697.5

下载: 导出CSV

表 2 控制器参数

Table 2. Parameters of controller

控制器	值
SNFTSMC	k₁=1、k₂=0.1、k₃=80、k₄=50、a=2.5、b=1.5、γ=1.5、λ=1、η=0.5、M=15
SMC	k₁=30、k₂=50、c=10、M=15

下载: 导出CSV

参考文献(22)

[1]	ZHENG S Q, WANG C. Rotor balancing for magnetically levitated TMPs integrated with vibration self-sensing of magnetic bearings[J]. IEEE/ASME Transactions on Mechatronics, 2021, 26(6): 3031-3039. doi: 10.1109/TMECH.2021.3051372
[2]	WANG K, MA X, LIU Q, et al. Multiphysics global design and experiment of the electric machine with a flexible rotor supported by active magnetic bearing[J]. IEEE/ASME Transactions on Mechatronics, 2019, 24(2): 820-831. doi: 10.1109/TMECH.2019.2892392
[3]	HUTTERER M, WIMMER D, SCHRÖDL M. Stabilization of a magnetically levitated rotor in the case of a defective radial actuator[J]. IEEE/ASME Transactions on Mechatronics, 2020, 25(6): 2599-2609. doi: 10.1109/TMECH.2020.2985623
[4]	DU L, CUI P L, ZHOU X X, et al. Unbalance vibration control for MSCMG based on high-precision synchronous signal detection method[J]. IEEE Sensors Journal, 2021, 21(16): 17917-17925. doi: 10.1109/JSEN.2021.3082695
[5]	刘贺. 主动磁悬浮轴承的H_∞控制研究[D]. 大连:大连理工大学,2021.
[6]	ZHANG X Y, CHEN X K, ZHU G Q, et al. Output feedback adaptive motion control and its experimental verification for time-delay nonlinear systems with asymmetric hysteresis[J]. IEEE Transactions on Industrial Electronics, 2020, 67(8): 6824-6834. doi: 10.1109/TIE.2019.2938460
[7]	王忠博,毛川,祝长生. 主动电磁轴承–刚性转子系统PID控制器设计方法[J]. 中国电机工程学报,2018,38(20): 6154-6163. WANG Zhongbo, MAO Chuan, ZHU Changsheng. A design method of PID controller for active magnetic bearings-rigid rotor systems[J]. Proceedings of the CSEE, 2018, 38(20): 6154-6163.
[8]	周天豪,杨智,祝长生,等. 电磁轴承高速电机转子系统的内模-PID控制[J]. 电工技术学报,2020,35(16): 3414-3425. ZHOU Tianhao, YANG Zhi, ZHU Changsheng, et al. Internal model control-PID control of an active magnetic bearing high-speed motor rotor system[J]. Transactions of China Electrotechnical Society, 2020, 35(16): 3414-3425.
[9]	MU C X, HE H B. Dynamic behavior of terminal sliding mode control[J]. IEEE Transactions on Industrial Electronics, 2018, 65(4): 3480-3490. doi: 10.1109/TIE.2017.2764842
[10]	任萍,朱景伟,赵燕,等. 基于双滑模控制器的开关磁阻电机调速策略[J]. 中国电机工程学报,2024,44(11): 4501-4513. REN Ping, ZHU Jingwei, ZHAO Yan, et al. Speed control strategy for switched reluctance motor based on dual sliding mode controller[J]. Proceedings of the CSEE, 2024, 44(11): 4501-4513.
[11]	HOU H Z, YU X H, XU L, et al. Finite-time continuous terminal sliding mode control of servo motor systems[J]. IEEE Transactions on Industrial Electronics, 2020, 67(7): 5647-5656. doi: 10.1109/TIE.2019.2931517
[12]	XU D Z, DING B, JIANG B, et al. Nonsingular fast terminal sliding mode control for permanent magnet linear synchronous motor via high-order super-twisting observer[J]. IEEE/ASME Transactions on Mechatronics, 2022, 27(3): 1651-1659. doi: 10.1109/TMECH.2021.3086527
[13]	李冰林,曾励,张鹏铭,等. 主动磁悬浮轴承的滑模自抗扰解耦控制[J]. 电机与控制学报,2021,25(7): 129-138. LI Binglin, ZENG Li, ZHANG Pengming, et al. Sliding mode active disturbance rejection decoupling control for active magnetic bearings[J]. Electric Machines and Control, 2021, 25(7): 129-138.
[14]	靖永志,冯伟,王森,等. 基于自适应非奇异终端滑模的悬浮控制策略[J]. 西南交通大学学报,2022,57(3): 566-573. doi: 10.3969/j.issn.0258-2724.20210743 JING Yongzhi, FENG Wei, WANG Sen, et al. Levitation control strategy based on adaptive non-singular terminal sliding mode[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 566-573. doi: 10.3969/j.issn.0258-2724.20210743
[15]	WANG J X, RONG J Y, YANG J. Adaptive fixed-time position precision control for magnetic levitation systems[J]. IEEE Transactions on Automation Science and Engineering, 2023, 20(1): 458-469. doi: 10.1109/TASE.2022.3156943
[16]	赵静,林智昌,姜斌,等. 永磁同步直线电机的分数阶超螺旋滑模控制[J]. 控制理论与应用,2023,40(7): 1224-1232. doi: 10.7641/CTA.2022.20162 ZHAO Jing, LIN Zhichang, JIANG Bin, et al. Fractional order super-twisting sliding mode control of permanent magnet synchronous linear motor[J]. Control Theory & Applications, 2023, 40(7): 1224-1232. doi: 10.7641/CTA.2022.20162
[17]	KALI Y, AYALA M, RODAS J, et al. Time delay estimation based discrete-time super-twisting current control for a six-phase induction motor[J]. IEEE Transactions on Power Electronics, 2020, 35(11): 12570-12580. doi: 10.1109/TPEL.2020.2995773
[18]	WANG J X, ZHAO L, YU L. Adaptive terminal sliding mode control for magnetic levitation systems with enhanced disturbance compensation[J]. IEEE Transactions on Industrial Electronics, 2021, 68(1): 756-766. doi: 10.1109/TIE.2020.2975487
[19]	东野亚兰,杨淑英,王奇帅,等. 基于增强型扩张状态观测器的永磁同步电机低抖振高抗扰二阶终端滑模电流控制[J]. 电工技术学报,2024,39(8): 2434-2448. DONGYE Yalan, YANG Shuying, WANG Qishuai, et al. Enhanced extended state observer based second order terminal sliding mode current control for permanent magnet synchronous machine with low chattering and improved disturbance rejection[J]. Transactions of China Electrotechnical Society, 2024, 39(8): 2434-2448.
[20]	朱良红,张国强,李宇欣,等. 基于级联扩张观测器的永磁电机无传感器自抗扰控制策略[J]. 电工技术学报,2022,37(18): 4614-4624. ZHU Lianghong, ZHANG Guoqiang, LI Yuxin, et al. Active disturbance rejection control for position sensorless permanent magnet synchronous motor drives based on cascade extended state observer[J]. Transactions of China Electrotechnical Society, 2022, 37(18): 4614-4624.
[21]	XU W, DIAN R J, LIU Y, et al. Robust flux estimation method for linear induction motors based on improved extended state observers[J]. IEEE Transactions on Power Electronics, 2019, 34(5): 4628-4640. doi: 10.1109/TPEL.2018.2865800
[22]	ZHANG T R, XU Z, GERADA C. A nonlinear extended state observer for sensorless IPMSM drives with optimized gains[J]. IEEE Transactions on Industry Applications, 2020, 56(2): 1485-1494. doi: 10.1109/TIA.2019.2959537

施引文献

附加材料(0)

访问统计

点击查看大图

图(18) / 表(2)

计量

文章访问数: 125
HTML全文浏览量: 109
PDF下载量: 14
被引次数: 0

1. 单自由度AMBs系统模型
2. SNFTSMC设计
2.1 SNFTSMC设计
2.2 稳定性分析
3. 考虑NESO的SNFTSMC设计
3.1 ESO设计
3.2 含NESO的SNFTSMC稳定性分析
4. 仿真分析
5. 实验验证
6. 结　论

主动磁悬浮轴承非奇异快速终端滑模转子位置控制

doi: 10.3969/j.issn.0258-2724.20240090

作者简介: 巩磊（1993—），男，讲师，研究方向为高速转子系统振动主动控制，E-mail：gong_lei@sust.edu.cn

计量

出版历程

Non-Singular Fast Terminal Sliding Mode Rotor Position Control of Active Magnetic Bearings

1. 单自由度AMBs系统模型

2. SNFTSMC设计

2.1 SNFTSMC设计

2.2 稳定性分析

3. 考虑NESO的SNFTSMC设计

3.1 ESO设计

3.2 含NESO的SNFTSMC稳定性分析

4. 仿真分析

5. 实验验证

6. 结 论

计量

出版历程

目录

1. 单自由度AMBs系统模型

2. SNFTSMC设计

2.1 SNFTSMC设计

2.2 稳定性分析

3. 考虑NESO的SNFTSMC设计

3.1 ESO设计

3.2 含NESO的SNFTSMC稳定性分析

4. 仿真分析

5. 实验验证

6. 结 论

作者简介:
巩磊（1993—），男，讲师，研究方向为高速转子系统振动主动控制，E-mail：gong_lei@sust.edu.cn

6. 结　论

6. 结　论