Review of Research on Vulnerability of Transportation Infrastructure to Extreme Climatic Conditions
-
摘要:
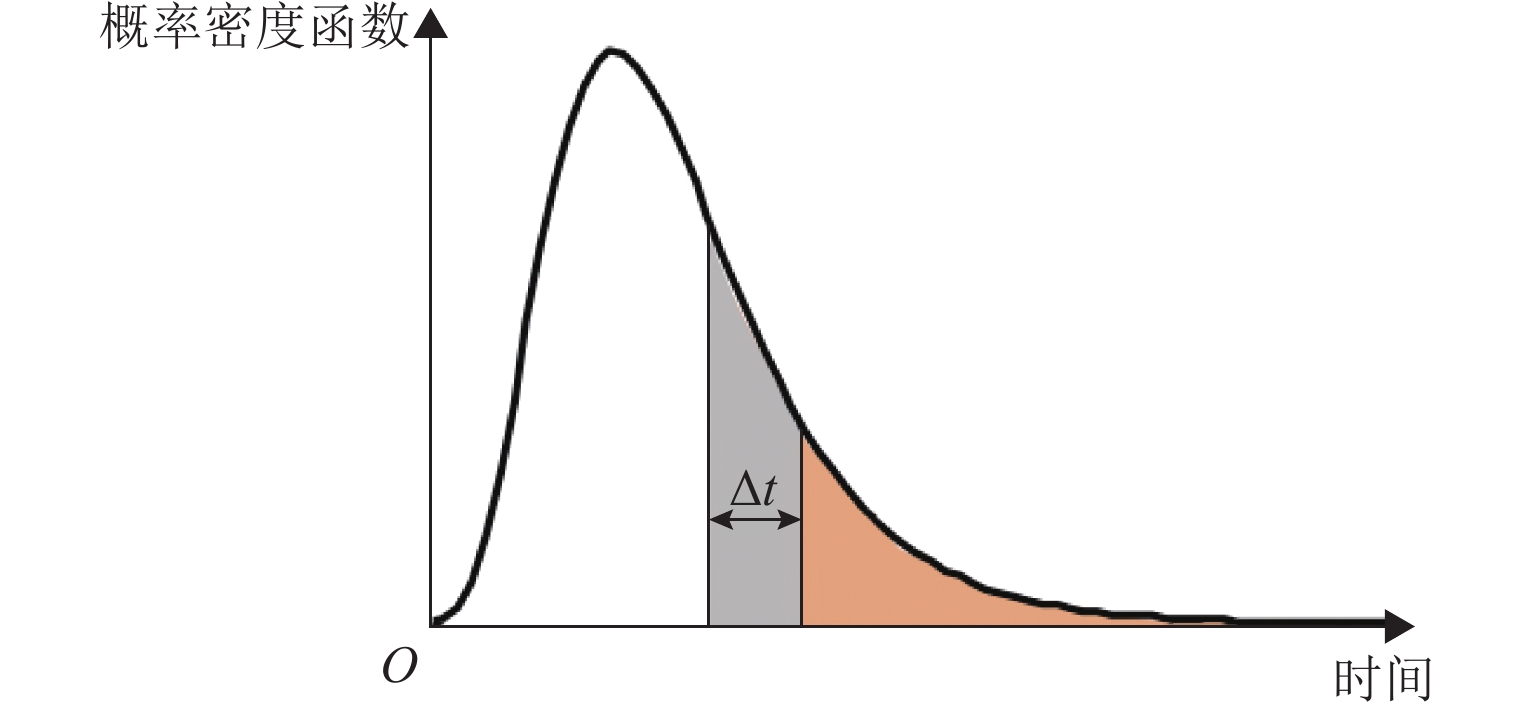
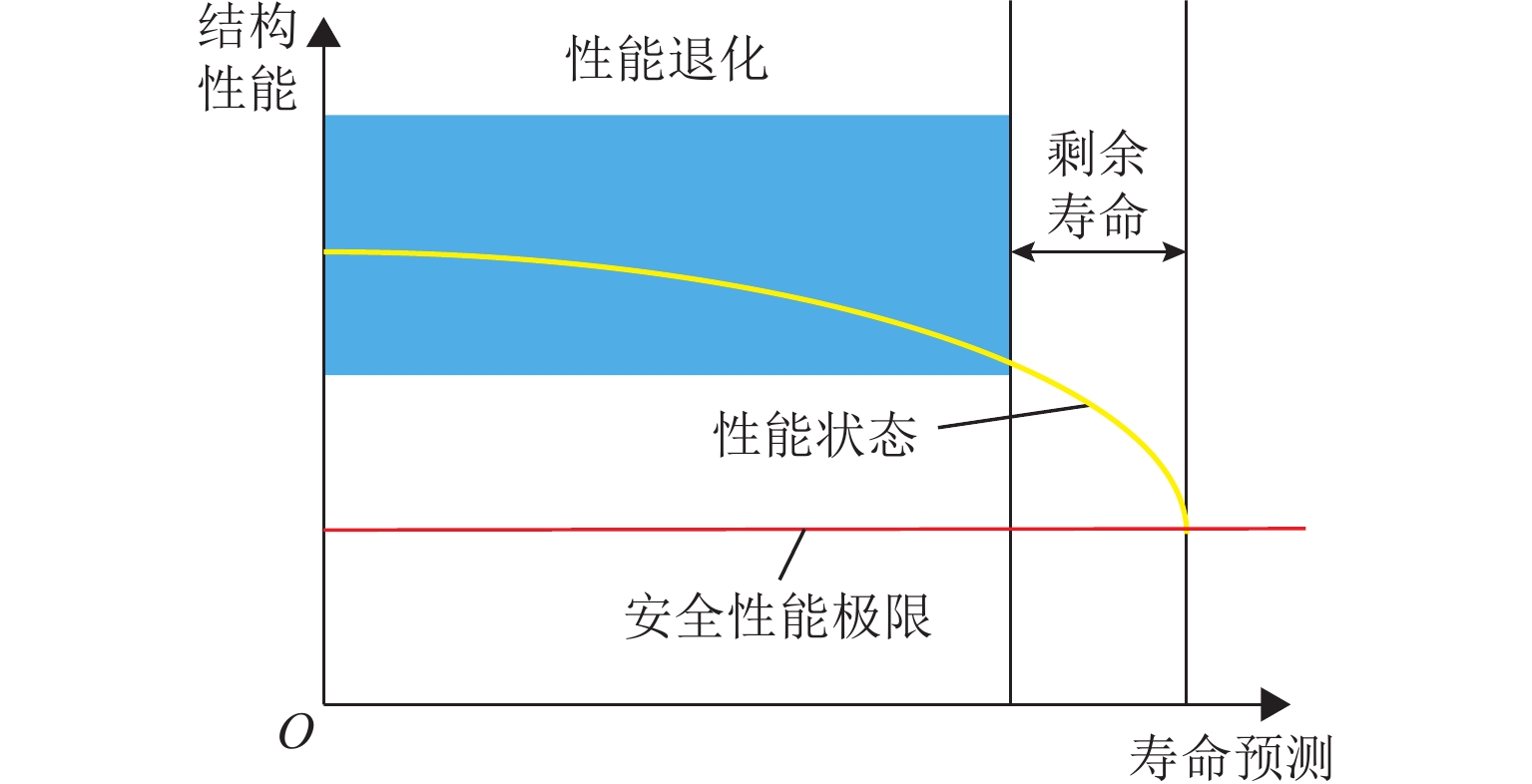
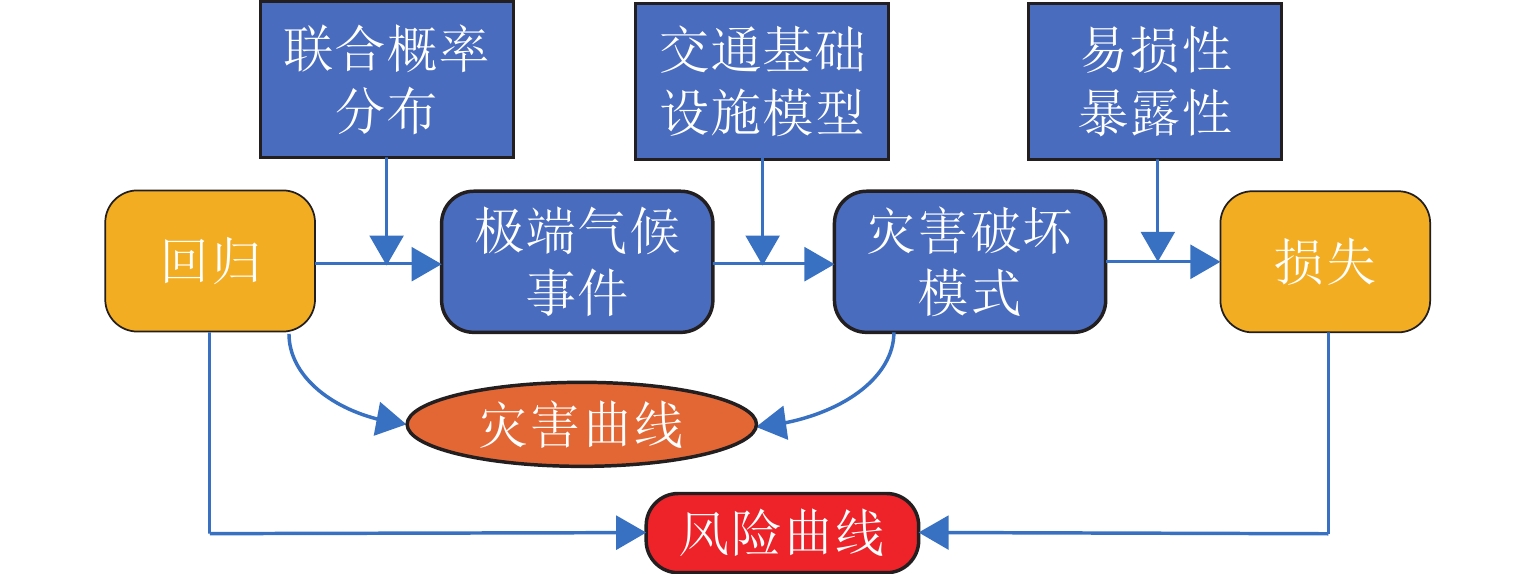
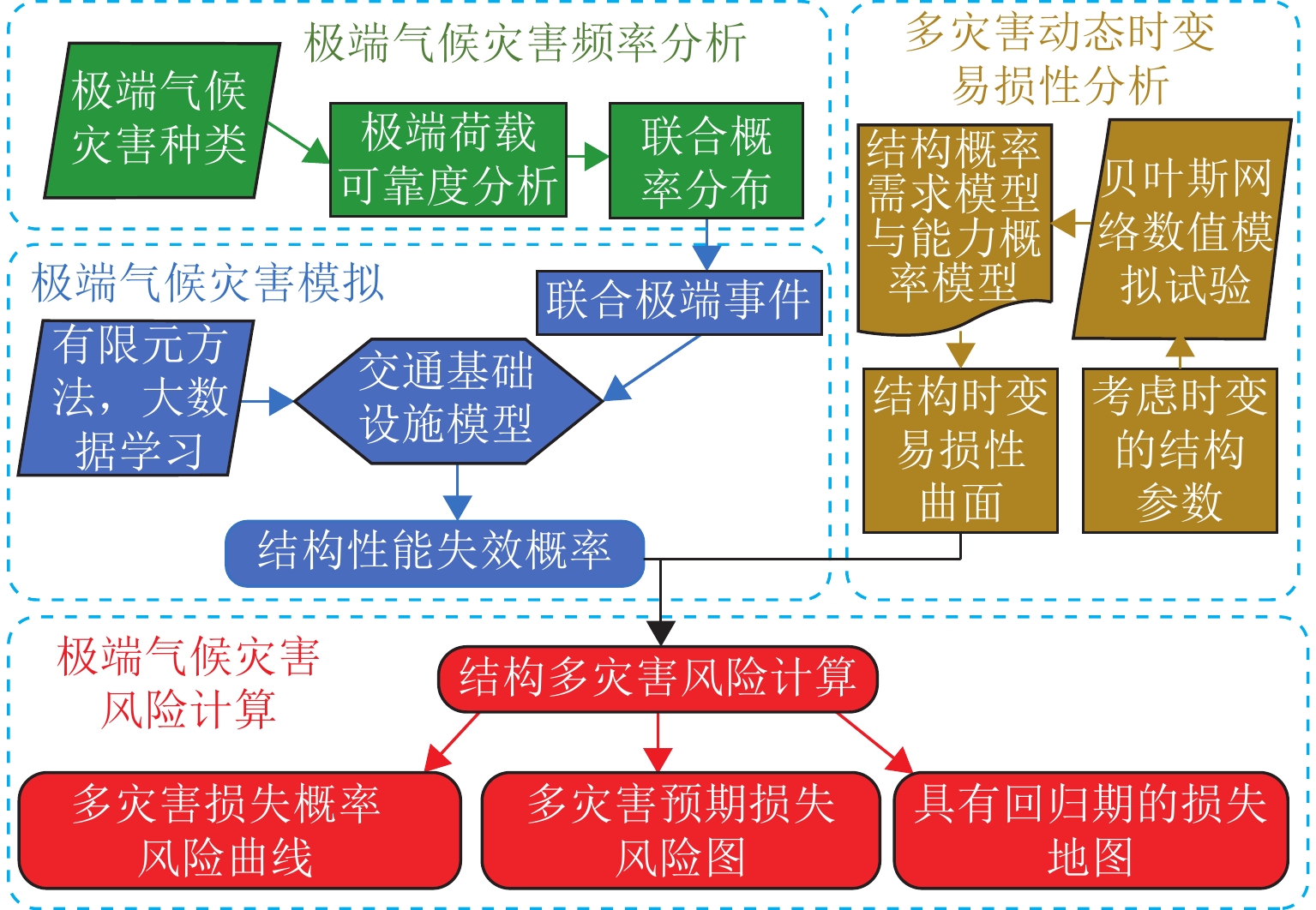
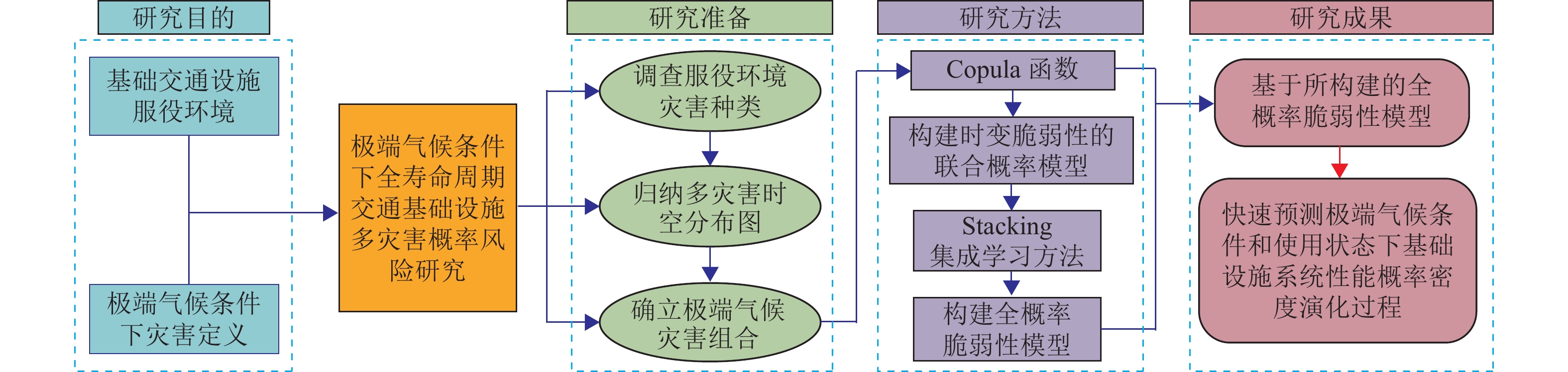
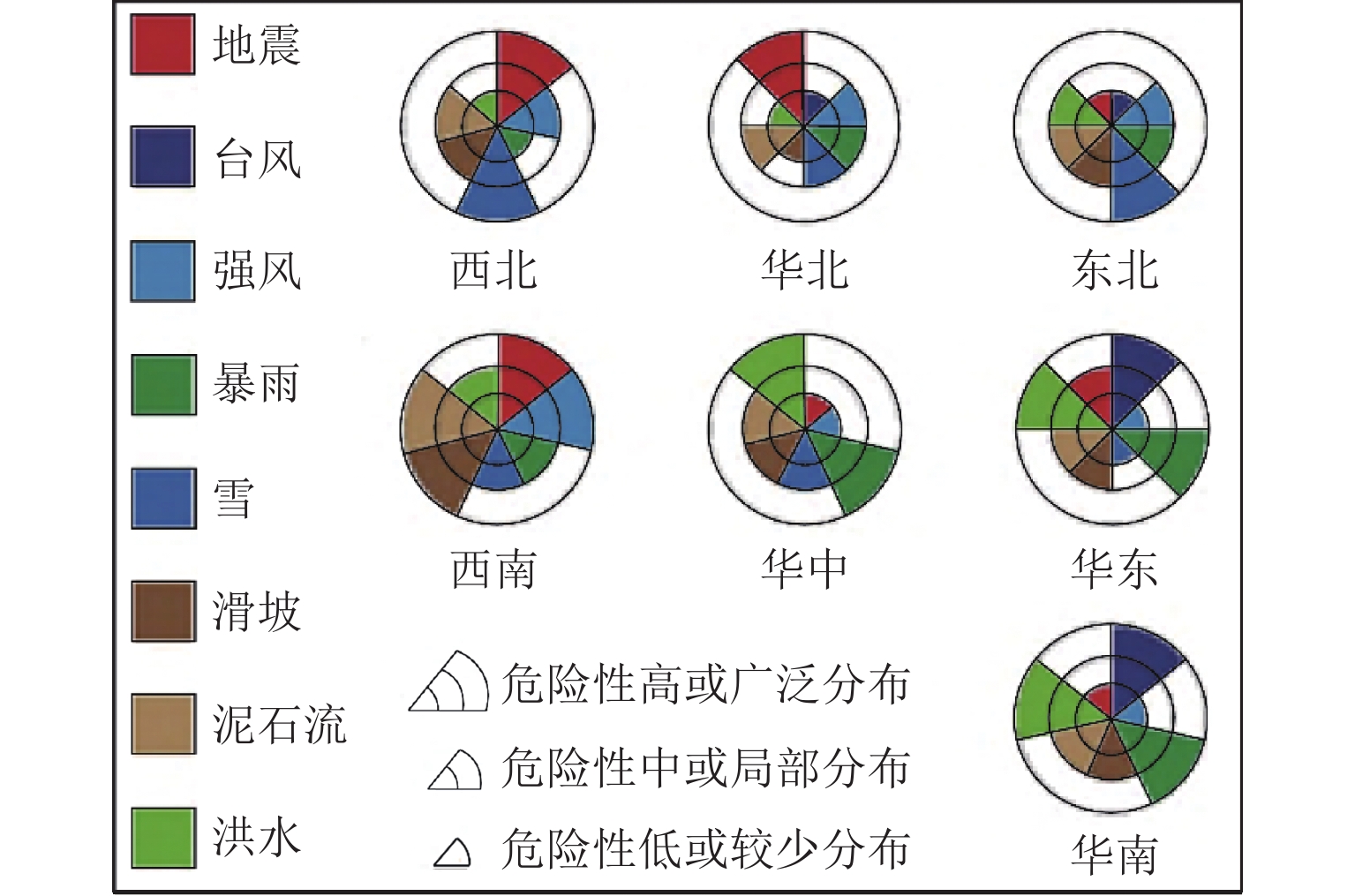
全球气候变化日益剧烈，极端强降水、高温、低温以及干旱等极端气候事件对现有交通基础设施的运行性能造成影响，甚至导致严重损坏. 与此同时，随着交通强国战略的深入实施，大量新的交通基础设施在恶劣环境中被建设，新建设施的功能性、耐久性和维护管理面临前所未有的挑战. 极端气候荷载变化迅速且难以预测，常常伴随多种灾害的耦合效应，使得交通基础设施在其作用下的破坏机理极为复杂. 为确保极端气候条件下交通基础设施的安全和效能，在国内外极端气候及多灾害耦合研究的基础上，系统梳理了极端气候的时空演变、多灾害耦合作用的研究历程以及多重灾害对工程结构的影响机理. 在此基础上，明确了极端气候影响的特性，并提出交通基础设施在设计、施工和维护阶段的防灾减灾设计原则. 同时，综合总结了在极端气候条件下交通基础设施的多灾害风险评估方法，并对未来的研究方向进行展望，指出利用人工智能和机器学习技术进行极端气候灾害的快速预测和评估，以及在全寿命周期内分析交通基础设施系统性能的变化将成为重要的发展趋势. 为桥梁、道路和隧道等交通基础设施在极端气候条件下的抗灾设计、性能评估和韧性提升提供了宝贵的参考.
Abstract:The intensifying global climate change is increasingly affecting the operational performance of existing transportation infrastructure due to extreme climatic events such as heavy precipitation, high temperatures, low temperatures, and drought, leading to severe damage. Meanwhile, with the further implementation of the strategy of building China with a strong transportation network, a significant number of new transportation infrastructure projects are being constructed in harsh environments, posing unprecedented challenges to the functionality, durability, and maintenance management of these new facilities. The characteristics of extreme climate loads include rapid and unpredictable variations, often accompanied by coupled effects of multiple disasters, rendering the mechanisms of damage to transportation infrastructure under their influence highly complex. To ensure the safety and effectiveness of transportation infrastructure under extreme climatic conditions, Chinese and international research on extreme climate and multi-disaster coupling was studied, and the research progress on spatiotemporal evolution of extreme climates and multi-disaster coupling effects was systematically reviewed. The impact mechanisms of multiple disasters on engineering structures were sorted out. Based on this foundation, the characteristics of extreme climate impacts were defined, and disaster prevention and reduction design principles for transportation infrastructure during the design, construction, and maintenance phases were proposed. Furthermore, methods for assessing multi-disaster risks to transportation infrastructure under extreme climatic conditions were comprehensively summarized, and future research was prospected, highlighting the importance of utilizing artificial intelligence and machine learning technologies for rapid prediction and assessment of extreme climatic disasters and analyzing changes in the performance of transportation infrastructure systems throughout their whole life cycle. This research provides valuable references for the disaster-resistant design, performance assessment, and resilience enhancement of transportation infrastructure such as bridges, roads, and tunnels under extreme climatic conditions.
-
Key words:
- extreme climate /
- transportation infrastructure /
- multi-disaster /
- whole life cycle /
- seismic resilience
-
在起重机械中薄壁箱形梁在工程中得到了广泛的使用，在这些应用中减轻重量是非常重要的. 同时，由于这种结构的特点是高刚度重量比，且在静载荷和动载荷的作用下薄壁箱形梁对扭转和不稳定性都非常敏感. 薄壁箱形梁在反对称荷载作用下，除引起截面扭转外，还产生截面畸变. 在薄壁箱形结构设计中，传统方法仅考虑延伸、弯曲、扭转、翘曲4种位移形变，在高阶模态下，若不考虑畸变效应，将大大影响自由振动频率预测的准确度. 为了提高薄壁箱形梁结构的计算精度，根据结构力学特征建立广义位移模型，在原有基础上，增加畸变形变，这对薄壁箱形梁振动特性的研究具有重要的意义.
Gere等[1]采用Rayleigh-Ritz方法研究了各种边界条件下均布梁的弯扭耦合自由振动. 根据圣维南理论，Dokumaci等[2-3]在静载荷和动载荷作用下建立了一些存有扭转的模型. Ohga等[4]利用传递矩阵法研究了开、闭截面薄壁构件的自由振动. 此外，Adam[5]分析了单对称梁的耦合弯扭振动. Arpaci等[6-7]研究了任意截面梁的自由振动. 对于任意开口截面薄壁梁的振动，Jrad等[8]推导了不同边界条件下弯扭耦合模态的高自由振动模态的解析解. Gökdağ等[9]研究了单对称开截面梁的自由振动和受迫振动的弯扭耦合. 在模型中，由虚功原理导出了包含质量和刚度特性的动力刚度矩阵. Rafezy等[10]建立了三维非对称截面的复合材料梁弯曲运动的精确动力刚度矩阵. 李海超等[11]提出一种半解析法来分析圆柱壳结构自由振动特性. 蒲育等[12]基于扩展的N阶广义剪切变形梁理论获得弹性地基简支梁的自由振动精确解. Ovesy等[13]通过考虑不同的本构方程假设，对薄壁组合梁的自由振动特性进行了研究. Vo等[14]利用精剪变形理论对任意叠合梁进行了振动和屈曲分析. 该理论解释了剪切应变随梁高的抛物线变化，产生的耦合称为三耦合振动和屈曲. Filippi等[15]提出了一类用于组合结构静力和动力分析的新型精细化梁理论. 这些梁模型是通过对梁截面上位移场的3个分量执行切比雪夫多项式的高阶展开得到的. 考虑轴向翘曲效应和材料各向异性对壳壁中表面剪切应变的影响，Wang等[16]提出了一种精度较高的薄壁封闭截面组合梁结构动力学模型.
虽然一些文献对薄壁闭合梁进行了动力分析，但很少有文献考虑弯曲、扭转和畸变的耦合来求解薄壁闭合梁. 分析发现，薄壁箱梁的截面畸变对固有频率有显著影响. 因此，要正确地预测薄壁箱梁的动力特性，必须同时考虑弯曲、扭转和畸变形变. Kim等[17]就薄壁箱梁静力和动力进行了翘曲、扭转和畸变耦合变形的静力和动力特性分析. Kaya等[18]采用微分变换方法（DTM）分析轴向加载的闭口截面复合材料Timoshenko梁的自由振动响应，该梁的材料耦合特性是由于铺层方向的影响而发生弯曲和扭转振动. Kim [19]对在轴向力作用下组合梁的空间耦合进行了自由振动分析，利用能量原理推导出运动方程和力-位移关系方程，并基于位移分量的幂级数展开式给出位移参数的显式表达式. 基于能量法，Prokić等[20]利用Benscoter理论推导了薄壁梁的微分运动方程，研究了简支薄壁梁的自由振动行为. 但仅考虑到横向剪切变形、转动惯量及翘曲影响，假设了薄壁箱形梁板面完全刚性，即忽略了箱梁横截面的畸变形变. Dancila等 [21]提出了除非考虑薄壁箱形梁横截面畸变形变，否则无法准确预测更高的固有频率.
本文基于弹性力学理论，用能量法分析了简支薄壁箱形梁的自由振动. 考虑到弯曲、扭转和畸变的耦合作用，预测了薄壁箱形梁自由振动的固有频率和模态振型. 根据Benscoter理论，在广义位移的基础上，将各变形模态用广义坐标位移表示出来，进而求出薄壁箱形梁的固有频率和模态振型的精确显示公式. 该表达式简单明了，适合于一般的简支薄壁箱形梁理论的实际使用.
1. 动力学基本理论
1.1 基本假设
为了确定薄壁箱形梁的几何形状，使用了两个坐标系. 如图1所示. 图中：θ为扭转角. (x，y，z)为笛卡尔直角坐标系，其中，x轴与薄壁箱形梁的梁长重合，y轴和z轴与横截面的主轴重合. (n，s，x)为局部坐标系，其中，n为沿等值线（截面中线）测量的法向坐标，s为任意起始点沿等值线测量的剖面坐标. 点M(w，v，u)为横截面上任意一点，其中，v、w、u分别为y、z、x轴方向的位移. 薄壁箱形梁的理论基于以下假设：
1） 在各板中，中线平面沿轮廓线方向的线剪切应变分布与圣维南扭转的线剪切应变分布相同；
2） 假设薄壁箱形梁截面的中面轮廓不可延伸；
3） 变形量足够小，可采用线弹性理论.
1.2 运动学方程
根据薄壁箱形梁的静力屈曲分析[22]可以得出，薄壁箱形梁上以任意一点M为初始点的位移分量在小扭转的情况下，使用Vlasov模型产生的经典位移关系为
ui(s,n,x,t)=u(x,t)+Zi(s)w′(x,t)+Yi(s)v′(x,t)−ωi(s)θ′(x,t)−Ωi(s)χ′(x,t), (1) vi(s,n,x,t)=−ξ1v(x,t)−ξ2w(x,t)−hi(s)θ(x,t)+ηi(s,n)χ(x,t), (2) wi(s,n,x,t)=−ξ1w(x,t)−ξ2v(x,t)−sθ(x,t)+ψni(s)χ(x,t), (3) 式中：χ为畸变角；i = 1 ~ 4是箱梁各板的编号；ωi为扭转翘曲函数，如式（4）；Ωi为畸变翘曲函数，如式（5）；ηi、ψni分别为横截面y轴和z轴方向的畸变位移，如式（6）、（8）；t为时间；Zi(s)、Yi(s)、hi(s)均为横截面系数，分别如式（9） ~ （11）；
$ \xi_{1} $ 、$ \xi_{2} $ 分别如式（12）、（13）.ωi(s)=[ξ22(b2+ξ2y0)−ξ21(h2+ξ2z0)]s, (4) Ωi(s)=∫s0ψsi(s)ds, (5) ηi(s,n)=ψsi(s)+n∂ψni(s)∂s, (6) ψsi(s)=dZi(s)dsYi(s)−dYi(s)dsZi(s), (7) ψni(s)=(−Lside2+3Lsides2−2L2sides3)(−1)i+1κ, (8) κ={2b/(b+h),i=1,3,2h/(b+h),i=2,4, Zi(s)=ξ2(h2+ξ1z0)+ξ2s, (9) Yi(s)=ξ2(b2+ξ2y0)−ξ1s, (10) hi(s)=ξ21(h2+ξ1z0)+ξ22(b2+ξ2y0), (11) ξ1=1+(−1)i+12(2−i)， (12) ξ2=1+(−1)i2(i−3)， (13) 式（4） ~ （13）中：b和h分别为箱梁横截面的宽和高；(z0, y0)为横截面的剪切中心；Lside为箱梁横截面各边相对应的长度.
根据Kim等[17]理论研究，横截面畸变形变的几何系数ψsi(s)可满足主要的静、动平衡条件. 此外，当每个板是不等厚时，κ是不连续的.
本文重点研究薄壁箱形梁在动载荷作用下的行为. 因此，在自由振动和线性动力学框架下，用式（1） ~ （3）作为运动学方程是充分、有效的. 为此需要对应变、动能和外载荷进行分析，进而推导运动控制方程. 应变能U的微分为
δU=4∑i=1∫∬(σxiδεxi+τsiδγsi)dFidx, (14) 式中：σxi和τsi为薄壁箱形梁的正应力和切应力分量；εxi和
$\gamma_{si} $ 分别为正应变和剪切应变，分别如式（15）、（16）；Fi为各板的面积；L为梁长.εxi=u′0+Zi(s)w″0+Yi(s)v″0−ωi(s)θ″−Ωi(s)χ″, (15) γsi=−(h′i(s)+∂ω′i(s))θ′+(η′i(s,n)+Ω′i(s))χ′, (16) 式中：u0、w0、v0分别为任意点M在u、w、v方向的初始位移.
应变能的变化可以用薄壁单元截面上的内力函数表示. 在动力学系统变分原理的基础上，推导动力学运动方程. 根据式（14）对横截面进行积分后，应变能可表示为
δU=∫L(Nδu′+Mzδv″−Myδw″+Bωθδθ″+Bωχδχ″+Msvδθ′+Msvχδχ′)dx, (17) 式中：N为轴向力；My和Mz分别为关于y轴和z轴的弯矩；Bωθ为双力矩；Bωχ为畸变引起的双力矩；Msv为圣维南扭矩；Msvχ为畸变引起的扭矩. 具体如式（18）所示.
{N=4∑i=1∬σxidFi,My=4∑i=1∬σxiZi(s)dFi,Mz=−4∑i=1∬σxiYi(s)dFi,Bωθ=−4∑i=1∬σxiωi(s)dFi,Bωχ=−4∑i=1∬σxiΩi(s)dFi,Msv=4∑i=1∬τxsi(hi(s)+∂ωi(s))dFi,Msvχ=4∑i=1∬τxsi(ηi(s,n)−Ω′i(s))dFi. (18) 2. 运动控制方程
在能量原理的基础上，应用哈密顿理论推导运动控制方程. 箱梁总能量的微分可由应变能U、动能T和外载荷功W表示，如式（19）.
δ∫t2t1(U−T−W)dt=0, (19) 式中：t1和t2分别为起、止时间.
利用虚位移原理可以得到薄壁箱形梁的运动方程. 图2中考虑了横截面x1=x和x2 = x + dx之间的一个小单元，在中面上承受单位面积上的外载荷p(px, py, pz)，其中：
${p_x}、\,{p_y}、\, {p_{\textit{z}}}$ 分别为x、y、z单位面积上的外载荷.图2中：ρ为单位体积质量密度. 在横截面上作意一点的应力为σ=−τxssinα+τxscosα+σx， (20) 式中：α为轮廓中线的法线与x轴之间的夹角；
${\tau _{xs}} $ 为切应力.虚位移δu满足必要的连续性条件和位移边界条件，可以采用与实位移向量相同的形式. 根据虚位移原理，虚位移可表示为
δu=δv+δw+δu=−ξ1iδw−ξ2iδv−sδθ+ψni(s)δχ+−ξ1iδv−ξ2iδw−hi(s)δθ+ηi(s,n)δχ+δu+zi(s)δw′+Yi(s)δv′−ωi(s)δθ′−Ωi(s)δχ′. (21) 加速度矢量可以表示为
δ¨u=δ¨v+δw⃛ (22) 等质量密度薄壁箱形梁单元的动能为
T = \frac{1}{2}{\int \iint {\rho \left[{{\left(\frac{{\partial u}}{{\partial t}}\right)}^2} + {{\left(\frac{{\partial v}}{{\partial t}}\right)}^2} + {{\left(\frac{{\partial w}}{{\partial t}}\right)}^2}\right]} } {\rm{d}}F_i{\rm{d}}x. (23) 动能的微分表示为
\text{δ} T = \rho \iint {\ddot u}\text{δ} u{\rm{d}}F_i. (24) 根据能量原理，薄壁箱形梁单元单位长度外载荷和惯性力的虚功为
\text{δ} W=\iint\left(\textit{σ}^{\prime} \text{δ} {u} + \sigma \text{δ} {u}^{\prime}\right) {\rm{d}} F_i + \int_{} \overline{{p}} \text{δ} {u} {{\rm{d}}} s, (25) 式中：
$\overline{{p}} $ 为惯性力.经整理计算，应用虚位移原理，可获得关于虚位移参数δu0、δv0、δw0、δθ、δχ的方程，对于任意虚位移参数均能恒等地满足这些方程，即虚位移参数的系数表达式必须消失. 将式（17）代入式（18），可以得到控制方程和相关的边界条件，则关于延伸、弯曲、扭转和畸变耦合的方程为
N^{\prime}-\rho \iint \ddot{u} \mathrm{ ~ d} F_i + p_{x}=0, (26) \left\{ \begin{array} {l} M_{{\textit{z}}}^{\prime \prime} + \rho \iint Y_i(s) u^{\prime} \mathrm{d} F_i + \rho \iint \ddot{v} \mathrm{ ~ d} F_i-p_{y} + m_{{\textit{z}}}^{\prime}=0,\\ M_{y}^{\prime \prime}-\rho \iint {\textit{Z}}_i(s) u^{\prime} \mathrm{d} F_i-\rho \iint \ddot{u} \mathrm{ ~ d} F_i + p_{{\textit{z}}} + m_{y}^{\prime}=0, \end{array} \right. (27) \begin{split} & \rho \iint \left\{ {\omega \frac{{{\partial ^2}u}}{{\partial {t^2}}}\frac{{\partial u}}{{\partial x}}} - {\left[({\textit{Z}}_i(s) - {{\textit{z}}_0})\ddot v - (Y_i(s) - {y_0})\ddot w\right]} \right\}{\rm{d}}F_i+ \\ & \quad {B''_{\omega \theta }}-{M'_{sv}} - {m_p} - {m_\omega } = 0, \end{split} (28) \begin{split} & \rho \iint{\left[\varOmega_i (s)\frac{{{\partial ^2}u}}{{\partial {t^2}}}\frac{{\partial u}}{{\partial x}}+{{\eta }_{i}}(s,n)\ddot{v}-\psi _{{\rm{n}}i}(s)\ddot{w}\right]}{\rm{d}}F_i+ \\ &\quad {{{{B}_{\omega \chi }''}}}-{{{{M}_{sv\chi }'}}}-{{m}_{p\chi }}-{{m}_{\varOmega }}=0 , \end{split} (29) 式中：mz和my分别为单位长度外力引起的关于z轴和y轴的弯矩；mp和mω分别为单位长度外力引起扭矩和双力矩；mpχ和mΩ分别为单位长度外力引起畸变扭矩和畸变双力矩.
式（26） ~ （30）是薄壁箱形梁延伸、弯曲、扭转和畸变的自由振动的最一般形式，变量u、v、w、θ、χ是完全耦合的.
对于具有连续质量分布且结构形式简单的结构可采用广义位移法. 对于简支梁，设任意时间t的任一截面的位移可用三角函数表示为
\left[\begin{array}{l} v(x, t)\\ w(x, t)\\ \theta(x, t)\\ \chi(x, t) \end{array}\right]=\left[\begin{array}{c} V(x)\\ W(x)\\ \varTheta(x)\\ X(x) \end{array}\right] \sin \;\omega t , (30) 式中：V(x)、W(x)、Θ(x)、Χ(x)分别为横向位移、纵向位移、扭转和畸变的振幅，如式（31）.
\left[ {\begin{array}{*{20}{c}} {V(x)} \\ {W(x)} \\ {\varTheta (x)} \\ {{\rm X}(x)} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{C_V}} \\ {{C_W}} \\ {{C_\varTheta }} \\ {{C_{X}}} \end{array}} \right]\sin\; {\lambda _k}, (31) 式中：
$\sin\; {\lambda _k} $ 为满足位移边界条件的振动模态函数，其中，${\lambda _k} $ =kπx/L，为形状函数, k=1，2，3，…；CV、CW、CΘ、CΧ均为常数.在简支梁的情况下，各支点处的支撑为防止旋转和可自由弯曲，其边界条件为
\left[\begin{array}{l} V(x) \\ W(x) \\ \varTheta(x) \\ X(x) \end{array}\right]=\left[\begin{array}{l} 0 \\ 0 \\ 0 \\ 0 \end{array}\right],\;\;\left[\begin{array}{l} V^{\prime \prime}(x) \\ W^{\prime \prime}(x) \\ \varTheta^{\prime \prime}(x) \\ X^{\prime \prime}(x) \end{array}\right]=\left[\begin{array}{l} 0 \\ 0 \\ 0 \\ 0 \end{array}\right]. (32) 为了求出CV、CW、CΘ、CΧ为非零解，式（30） ~ （32）的导数必须为0，即
\left[ {\begin{array}{*{20}{c}} {{I_y}\lambda _{{k}}^4 - {\omega _*}(\lambda _{k}^2{I_y} + A)}&0&{ - {{\textit{z}}_0}A{\omega _*}}&{{A_1}{\omega _*}} \\ 0&\begin{gathered} {I_{\textit{z}}}\lambda _{{k}}^4 \\ - {\omega _*}(\lambda _{{k}}^2{I_{\textit{z}}} + A) \\ \end{gathered} &{{y_0}A{\omega _*}}&{ - {A_2}{\omega _*}} \\ { - {y_0}A({I_{\omega \theta }}\lambda _{{k}}^2 + 1){\omega _*}}&{{{\textit{z}}_0}A({I_{\omega \theta }}\lambda _{{k}}^2 + 1){\omega _*}}&{ {I_{\omega \theta }}\lambda _{{k}}^4 + G/E{I_{\rm{t}}}\lambda _{{k}}^2 + ({I_{\rm{p}}} - {I_{\omega \theta }}\lambda _{{k}}^2){\omega _*} }&0 \\ {({A_1} + {A_3}\lambda _{{k}}^2){\omega _*}}&{ - ({A_2} + {A_4}\lambda _{{k}}^2){\omega _*}}&0&{ {I_{\omega \chi }}\lambda _{{k}}^4 + G/E{I_{\rm{d}}}\lambda _{{k}}^2 + ({I_{{\rm{p}}\chi }} - {I_{\omega \chi }}\lambda _{{k}}^2){\omega _*} } \end{array}} \right] = 0 , (33) 式中：
${\omega _ * } = \dfrac{\rho }{E}{\omega ^2}$ ，E为弹性模量；G为剪切模量；Iy和Iz分别为关于y、z轴的惯性矩；It和Iωθ分别为圣维南扭转和翘曲常数；Id和Iωχ分别为畸变引起的扭转和翘曲常数；Ip为扭转和翘曲耦合惯性矩；Ipχ为畸变与翘曲耦合惯性矩；A为横截面面积；A1、A2分别为纵向、横向弯曲与畸变耦合惯性矩参数；A3、A4分别为纵向、横向弯曲与翘曲耦合惯性矩参数.经整理可得四阶频率代数方程为
a \omega_{*}^{4} + c \omega_{*}^{3} + d \omega_{*}^{2} + e\omega_{*} + f=0 \text{，} (34) 式中：a、c、d、e和f均为常数.
3. 算例分析
3.1 畸变模态下薄壁箱形梁的自由振动（算例1）
为了验证理论公式（式（34））的正确性，求解薄壁箱形梁在畸变模态下自由振动时的频率和振型，并与有限元分析（ANSYS）结果进行对比. 图3为简支薄壁箱形梁的几何参数. 通过对薄壁箱梁的模态分析，验证该方法的有效性和准确性. 该梁的材料参数和几何参数为：E = 210 GPa，G = 80.7 GPa，ρ = 7 850 kg/m3，b = 150 mm，h = 200 mm. 薄壁箱形梁的板厚为：m1 = m3 = 4 mm，m2 = 8 mm，m4 = 5 mm. 在有限元分析过程中，ANSYS软件中采用4node181的壳单元进行分析，在网格划分后生成1932个节点和1890个单元.
表1为考虑横截面畸变形变的理论方法和有限元分析方法下薄壁箱形梁其中的4阶模态（2、4、6、8阶）的固有频率对比. 为了对结果进行评估，将有限元法的固有频率表示为ωFEA，本方法的固有频率表示为ω1, Δ为本理论的固有频率与有限元固有频率的相对误差.
表 1 薄壁箱形梁其中4阶的固有频率比较Table 1. Comparison of 2-, 4-, 6-, and 8-order natural frequencies of thin-walled box beams阶数 ω1/Hz ωFEA/Hz Δ/% 2 9.36 9.34 0.18 4 36.99 36.90 0.25 6 54.30 54.11 0.36 8 89.46 88.67 0.89 由表1可以看出：固有频率结果具有较强的一致性，其中最大误差为0.89%（在第8阶时），验证了本文分析方法可以较准确地进行薄壁箱形梁固有频率的预测；伴随着模态阶数的升高，固有频率的误差逐渐增加.
图4为2、4、6阶和8阶的弯曲模态振型.
由图4可以看出：薄壁箱形梁的所有振型均为傅立叶变换，所得到的弯曲模态振型与ANSYS弯曲模态振型结果吻合良好.
3.2 不同长度对自由振动的影响（算例2）
为了研究畸变形变对薄壁箱梁自由振动特性的影响，考虑了3种不同长度的结构. 将本文的计算结果与Prokić等[20]的弯扭耦合（不考虑畸变形变）情况下求解的自由振动的精确解进行对比分析. 薄壁箱形梁的材料参数和几何参数与算例1相同. 表2为箱梁长度分别为3、4、5 m时薄壁箱形梁的前4阶自由振动频率及其相对误差. Prokić方法计算的弯扭组合结果用ω1表示；考虑畸变形变的相关结果用ω2表示；Δ1为Prokić 结果与有限元分析法的自由振动频率的相对误差；Δ2为考虑畸变形变情况下的自由振动频率与有限元分析法的自由振动频率的相对误差.
表 2 简支薄壁箱形梁的固有频率Table 2. Natural frequencies of simply supported thin-walled box beamsL/m 阶数 ω1/Hz ω2/Hz ωFEA/Hz Δ1/% Δ2/% 3 1 11.92 11.91 11.91 0.06 0 2 63.16 63.12 63.03 0.20 0.14 3 64.90 64.08 64.67 0.35 0.20 4 114.41 114.36 113.93 0.42 0.38 4 1 7.33 7.33 7.33 0 0 2 36.87 36.86 36.83 0.10 0.08 3 50.04 50.01 49.91 0.26 0.20 4 76.63 76.57 76.34 0.38 0.30 5 1 5.11 5.11 5.11 0 0 2 25.79 25.79 25.77 0.08 0.06 3 35.72 35.71 35.64 0.23 0.19 4 61.68 61.64 61.47 0.34 0.28 在表2 中，将ω1与ωFEA进行比较，可以看出：本方法的解与有限元方法的结果有较强的一致性，且准确性和有效性较高，尤其是在第4阶模态情况下，最大相对误差Δ2仅为0.38%（当L=3 m）；与Prokić 方法相比，自由振动频率ω1 < ω2，即考虑畸变形变可有效降低自由振动频率，进而提高了自由振动数值精确解的有效值；长细比越大，自振频率越小；在高阶模态下考虑畸变时的固有频率更接近于有限元法的解，因此，在高阶模态上考虑畸变效应的振动分析更占优势.
4. 结 论
考虑畸变效应的耦合位移模态特征，提高了预测自由振动的准确度，有利于薄壁箱形梁的设计和性能分析. 得到如下结论：
1） 推导出包含轴向延伸、弯曲、翘曲、扭转和畸变的运动学高耦合强度的控制方程.
2） 考虑畸变形变的模态振型与有限元分析结果吻合较好. 前4阶模态情况下最大相对误差仅有0.38%（当L=3 m时）.
3） 与Prokić方法相比，考虑畸变效应情况下，自由振动频率可以得到有效降低，提高了自由振动数值精确解的有效值.
-
图 2 极端气候对交通基础设施的影响
Figure 2. Impacts of extreme climates on transportation infrastructure
图 6 暴雨导致Hatchie River 桥梁冲刷破坏
Figure 6. Erosion damage to Hatchie River Bridge caused by heavy rain
图 8 特大暴雨引起石太铁路桥梁垮塌
Figure 8. Collapse of Shijiazhuang-Taiyuan Railway Bridge caused by heavy rain
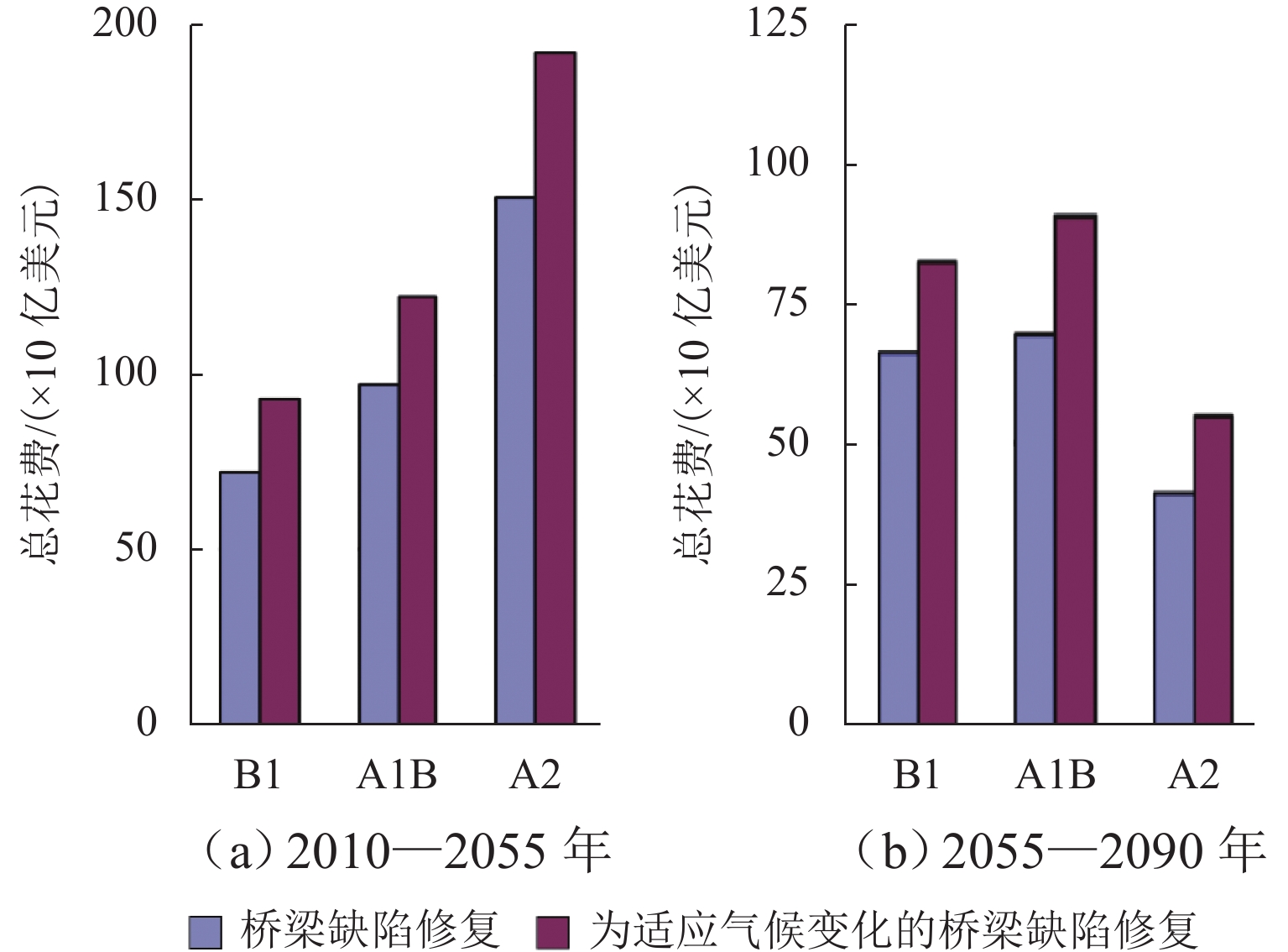
表 1 未来中国年平均地表气温与降水量（相对1961—1990年平均值）
Table 1. Annual average surface temperature and precipitation in China in the future （relative to average value in 1961–1990）
年份 温度变化/℃ 降水变化% 2020 年 1.3～2.1 2～3 2030 年 1.5～2.8 2050 年 2.3～3.3 5～7 2100 年 3.9～6.0 11～17 
下载: 导出CSV
-
[1] 丁一汇,任国玉,石广玉,等. 气候变化国家评估报告(Ⅰ): 中国气候变化的历史和未来趋势[J]. 气候变化研究进展,2006,2(1): 3-8.DING Yihui, REN Guoyu, SHI Guangyu, et al. National assessment report of climate change (Ⅰ): climate change in China and its future trend[J]. Climate Change Research, 2006, 2(1): 3-8. [2] 杨肖丽,马慧君,吴凡,等. 基于CMIP6的全球及干旱带干旱时空演变[J]. 水资源保护,2023,39(2):40-49.YANG Xiaoli, MA Huijun, WU Fan, et al. Spatiotemporal evolution of global and arid zone drought based on CMIP6[J]. Water Resources Protection, 2023, 39(2):40-49. [3] HU Q, TANG Z H, ZHANG L, et al. Evaluating climate change adaptation efforts on the US 50 states’ hazard mitigation plans[J]. Natural Hazards, 2018, 92(2):783-804. [4] WRIGHT L, CHINOWSKY P, STRZEPEK K, et al. Estimated effects of climate change on flood vulnerability of U. S. bridges[J]. Mitigation and Adaptation Strategies for Global Change, 2012, 17(8): 39-955. [5] 张存杰,肖潺,李帅,等. 极端气候事件综合危险性等级指标构建及近60年来长江流域极端气候综合分析[J]. 地球物理学报,2023,66(3): 920-938. doi: 10.6038/cjg2022Q0255ZHANG Cunjie, XIAO Chan, LI Shuai, et al. Construction of multi-extreme climate events composite grads index and comprehensive analysis of extreme climate in the Yangtze River Basin from 1961 to 2020[J]. Chinese Journal of Geophysics, 2023, 66(3): 920-938. doi: 10.6038/cjg2022Q0255 [6] TORRESAN S, CRITTO A, RIZZI J, et al. Assessment of coastal vulnerability to climate change hazards at the regional scale: the case study of the North Adriatic Sea[J]. Natural Hazards and Earth System Sciences, 2012, 12(7): 2347-2368. doi: 10.5194/nhess-12-2347-2012 [7] BARBATO M, PETRINI F, UNNIKRISHNAN V U, et al. Performance-Based Hurricane Engineering (PBHE) framework[J]. Structural Safety, 2013, 45: 24-35. doi: 10.1016/j.strusafe.2013.07.002 [8] 张喜刚,田雨,陈艾荣. 多灾害作用下桥梁设计方法研究综述[J]. 中国公路学报,2018,31(9): 7-19. doi: 10.3969/j.issn.1001-7372.2018.09.002ZHANG Xigang, TIAN Yu, CHEN Airong. Review of bridge design method for multiple hazards[J]. China Journal of Highway and Transport, 2018, 31(9): 7-19. doi: 10.3969/j.issn.1001-7372.2018.09.002 [9] GALLINA V, TORRESAN S, CRITTO A, et al. A review of multi-risk methodologies for natural hazards: consequences and challenges for a climate change impact assessment[J]. Journal of Environmental Management, 2016, 168: 123-132. [10] 李宏男,李钢,郑晓伟,等. 工程结构在多灾害耦合作用下的研究进展[J]. 土木工程学报,2021,54(5): 1-14.LI Hongnan, LI Gang, ZHENG Xiaowei, et al. Research progress in engineering structures subject to multiple hazards[J]. China Civil Engineering Journal, 2021, 54(5): 1-14. [11] 王元丰,韩冰. 极端气候事件对桥梁安全性的影响分析[J]. 土木工程学报,2009,42(3): 76-80. doi: 10.3321/j.issn:1000-131X.2009.03.013WANG Yuanfeng, HAN Bing. Influences of extreme climate events on bridges[J]. China Civil Engineering Journal, 2009, 42(3): 76-80. doi: 10.3321/j.issn:1000-131X.2009.03.013 [12] 王芸,赵鹏祥. 黄河流域极端气候事件的时空变异特征研究[J]. 西北林学院学报,2021,36(3): 190-196. doi: 10.3969/j.issn.1001-7461.2021.03.28WANG Yun, ZHAO Pengxiang. Temporal and spatial variation characteristics of extreme climate events in the Yellow River Basin[J]. Journal of Northwest Forestry University, 2021, 36(3): 190-196. doi: 10.3969/j.issn.1001-7461.2021.03.28 [13] CHOWDHURY M A, ZZAMAN R U, TARIN N J, et al. Spatial variability of climatic hazards in Bangladesh[J]. Natural Hazards, 2022, 110(3): 2329-2351 doi: 10.1007/s11069-021-05039-3 [14] HUSSAIN M A, ZHANG S, MUNEER M, et al. Assessing and mapping spatial variation characteristics of natural hazards in Pakistan[J]. Land, 2022, 12(1): 1-40. doi: 10.3390/land12010001 [15] ALEXANDER L V, ZHANG X, PETERSON T C, et al. Global observed changes in daily climate extremes of temperature and precipitation[J]. Journal of Geophysical Research: Atmospheres, 2006, 111: D05109.1-D05109.22 [16] KUNKEL K E, KARL T R, EASTERLING D R, et al. Probable maximum precipitation and climate change[J]. Geophysical Research Letters, 2013, 40(7): 1402-1408. doi: 10.1002/grl.50334 [17] FISCHER E M, SIPPEL S, KNUTTI R. Increasing probability of record-shattering climate extremes[J]. Nature Climate Change, 2021, 11: 689-695. doi: 10.1038/s41558-021-01092-9 [18] MARTINEZ-VILLALOBOS C, NEELIN J D. Regionally high risk increase for precipitation extreme events under global warming[J]. Scientific Reports, 2023, 13: 5579.1-5579.14. [19] 曹永旺,延军平. 1961—2013年山西省极端气候事件时空演变特征[J]. 资源科学,2015,37(10): 2086-2098.CAO Yongwang, YAN Junping. Temporal and spatial analysis of extreme climatic events in Shanxi Province from 1961 to 2013[J]. Resources Science, 2015, 37(10): 2086-2098. [20] 曹永强,袁立婷,郑爽,等. 近50年辽宁省极端气候事件的趋势变化及空间特征[J]. 水利水电技术,2018,49(7): 45-53.CAO Yongqiang, YUAN Liting, ZHENG Shuang, et al. Trend variation and spatial characteristics of extreme climate events in Liaoning Province in recent 50 years[J]. Water Resources and Hydropower Engineering, 2018, 49(7): 45-53. [21] 柴素盈,曹言,窦小东,等. 1964—2017年南盘江流域主要极端气候事件时空演变特征[J]. 水土保持研究,2020,27(1): 151-160.CHAI Suying, CAO Yan, DOU Xiaodong, et al. Analysis temporal and spatial changes of extreme climatic events in nanpan river basin from 1964 to 2017[J]. Research of Soil and Water Conservation, 2020, 27(1): 151-160. [22] 杨晨,董晓华,董立俊,等. 雅砻江流域1961—2018年极端气候时空演变研究[J]. 中国农村水利水电,2023(2): 46-56,65.YANG Chen, DONG Xiaohua, DONG Lijun, et al. Research on the temporal and spatial evolution of extreme climate in the yalong river basin from 1961 to 2018[J]. China Rural Water and Hydropower, 2023(2): 46-56,65. [23] BRUNETTI M, MAUGERI M, NANNI T. Changes in total precipitation, rainy days and extreme events in northeastern Italy[J]. International Journal of Climatology, 2001, 21(7): 861-871. doi: 10.1002/joc.660 [24] BOO K O, KWON W T, BAEK H J. Change of extreme events of temperature and precipitation over Korea using regional projection of future climate change[J]. Geophysical Research Letters, 2006, 33: L01701.1-L01701.4 [25] STEVENS L E, MAYCOCK T K, STEWART B C. Climate change in the human environment: indicators and impacts from the Fourth National Climate Assessment[J]. Journal of the Air & Waste Management Association, 2021, 71(10):1210-1233. [26] ARNELL N W, GOSLING S N. The impacts of climate change on river flood risk at the global scale[J]. Climatic Change, 2016, 134(3): 387-401. doi: 10.1007/s10584-014-1084-5 [27] FREI C, SCHÖLL R, FUKUTOME S, et al. Future change of precipitation extremes in Europe: Intercomparison of scenarios from regional climate models[J]. Journal of Geophysical Research: Atmospheres, 2006, 111: D06105.1-D06105.22. [28] HAHN M B, RIEDERER A M, FOSTER S O. The livelihood vulnerability index: a pragmatic approach to assessing risks from climate variability and change—a case study in Mozambique[J]. Global Environmental Change, 2009, 19(1): 74-88. doi: 10.1016/j.gloenvcha.2008.11.002 [29] ROBINE J M, CHEUNG S L K, LE ROY S, et al. Death toll exceeded 70, 000 in Europe during the summer of 2003[J]. Comptes Rendus Biologies, 2008, 331(2): 171-178. [30] STEWART S R. Eastern north pacific hurricanes 2010: flooding in a slow season[J]. Weatherwise, 2011, 64(3): 38-45. doi: 10.1080/00431672.2011.566819 [31] 王志军,李福普. 基于风险矩阵的极端气候因素对沥青混凝土路面的影响分析[J]. 公路,2014,59(7): 56-60.WANG Zhijun, LI Fupu. Analysis of impact on extreme climate factors to asphalt pavement based on risk matrix[J]. Highway, 2014, 59(7): 56-60. [32] 王林波. 极端气候对城市道路路面结构影响分析[J]. 内蒙古公路与运输,2018(1): 40-42.WANG Linbo. Analysis of the influence of extreme climate on urban road pavement structure[J]. Highways & Transportation in Inner Mongolia, 2018(1): 40-42. [33] BIXLER R P, YANG E, RICHTER S M, et al. Boundary crossing for urban community resilience: a social vulnerability and multi-hazard approach in Austin, Texas, USA[J]. International Journal of Disaster Risk Reduction, 2021, 66: 102613.1-102613.9. [34] WARD P J, BLAUHUT V, BLOEMENDAAL N, et al. Review article: natural hazard risk assessments at the global scale[J]. Natural Hazards and Earth System Sciences, 2020, 20(4): 1069-1096. doi: 10.5194/nhess-20-1069-2020 [35] ZHANG W, VILLARINI G. Deadly compound heat stress-flooding hazard across the central United States[J]. Geophysical Research Letters, 2020, 47(15): 1-7. [36] TEPPER F. The Sendai framework for disaster risk reduction and persons with disabilities[J]. International Journal of Disaster Risk Science, 2015, 6(2): 140-149. doi: 10.1007/s13753-015-0051-8 [37] SANTINI M, CACCAMO G, LAURENTI A, et al. A multi-component GIS framework for desertification risk assessment by an integrated index[J]. Applied Geography, 2010, 30(3): 394-415. doi: 10.1016/j.apgeog.2009.11.003 [38] LIANG Z, LEE G C. Bridge pier failure probabilities under combined hazard effects of scour, truck and earthquake. part I: occurrence probabilities[J]. Earthquake Engineering and Engineering Vibration, 2013, 12(2): 229-240. doi: 10.1007/s11803-013-0166-0 [39] LIANG Z, LEE G C. Towards establishing practical multi-hazard bridge design limit states[J]. Earthquake Engineering and Engineering Vibration, 2013, 12(3): 333-340. doi: 10.1007/s11803-013-0175-z [40] KAPPES M S, KEILER M, VON ELVERFELDT K, et al. Challenges of analyzing multi-hazard risk: a review[J]. Natural Hazards, 2012, 64(2): 1925-1958. doi: 10.1007/s11069-012-0294-2 [41] CHEN L, SINGH V P, GUO S L, et al. Flood coincidence risk analysis using multivariate copula functions[J]. Journal of Hydrologic Engineering, 2012, 17(6): 742-755. doi: 10.1061/(ASCE)HE.1943-5584.0000504 [42] 秦佩瑶. 地震与洪水联合作用下结构抗多灾分析与设防水准研究[D]. 大连:大连理工大学,2021. [43] SHIEH C L, CHEN Y S, TSAI Y J, et al. Variability in rainfall threshold for debris flow after the Chi-Chi earthquake in central Taiwan, China[J]. International Journal of Sediment Research, 2009, 24(2): 177-188. doi: 10.1016/S1001-6279(09)60025-1 [44] XU L F, MENG X W, XU X G. Natural hazard chain research in China: a review[J]. Natural Hazards, 2014, 70(2): 1631-1659. doi: 10.1007/s11069-013-0881-x [45] 门可佩,高建国. 重大灾害链及其防御[J]. 地球物理学进展,2008,23(1): 270-275.MEN Kepei, GAO Jianguo. Severe disaster chain and its defense[J]. Progress in Geophysics, 2008, 23(1): 270-275. [46] 周靖,马石城,赵卫锋. 城市生命线系统暴雪冰冻灾害链分析[J]. 灾害学,2008,23(4): 39-44. doi: 10.3969/j.issn.1000-811X.2008.04.009ZHOU Jing, MA Shicheng, ZHAO Weifeng. Analysis on disaster chains of urban lifeline system in heavy snow-freezing weather[J]. Journal of Catastrophology, 2008, 23(4): 39-44. doi: 10.3969/j.issn.1000-811X.2008.04.009 [47] KOMENDANTOVA N, MRZYGLOCKI R, MIGNAN A, et al. Multi-hazard and multi-risk decision-support tools as a part of participatory risk governance: feedback from civil protection stakeholders[J]. International Journal of Disaster Risk Reduction, 2014, 8: 50-67. doi: 10.1016/j.ijdrr.2013.12.006 [48] MARASCO S, ZAMANI NOORI A, CIMELLARO G P. Cascading hazard analysis of a hospital building[J]. Journal of Structural Engineering, 2017, 143(9): 04017100.1-04017100.15. [49] GILL J C, MALAMUD B D. Reviewing and visualizing the interactions of natural hazards[J]. Reviews of Geophysics, 2014, 52(4): 680-722. doi: 10.1002/2013RG000445 [50] GIDARIS I, PADGETT J E, BARBOSA A R, et al. Multiple-hazard fragility and restoration models of highway bridges for regional risk and resilience assessment in the United States: state-of-the-art review[J]. Journal of Structural Engineering, 2017, 143(3): 04016188.1-04016188.17. [51] TAYLOR Z, STOYANOFF S, DALLAIRE P O, et al. Aerodynamics of long-span bridges: susceptibility to snow and ice accretion[J]. Journal of Structural Engineering, 2017, 143(7): 04017039.1-04017039.11. [52] CHOE D E, GARDONI P, ROSOWSKY D. Fragility increment functions for deteriorating reinforced concrete bridge columns[J]. Journal of Engineering Mechanics, 2010, 136(8): 969-978. doi: 10.1061/(ASCE)EM.1943-7889.0000147 [53] GHOSH J, PADGETT J E. Aging considerations in the development of time-dependent seismic fragility curves[J]. Journal of Structural Engineering, 2010, 136(12): 1497-1511. doi: 10.1061/(ASCE)ST.1943-541X.0000260 [54] DONG Y, FRANGOPOL D M. Probabilistic time-dependent multihazard life-cycle assessment and resilience of bridges considering climate change[J]. Journal of Performance of Constructed Facilities, 2016, 30(5): 04016034.1-04016034.12. [55] SALMAN A M, LI Y. Multihazard risk assessment of electric power systems[J]. Journal of Structural Engineering, 2017, 143(3): 04016198.1-04016198.14. [56] 孔锋. 透视大尺度综合自然灾害风险评估的主要进展和展望[J]. 灾害学,2020,35(2): 148-153. doi: 10.3969/j.issn.1000-811X.2020.02.027KONG Feng. Perspective on the main progress and prospect of large-scale comprehensive natural disaster risk assessment[J]. Journal of Catastrophology, 2020, 35(2): 148-153. doi: 10.3969/j.issn.1000-811X.2020.02.027 [57] MARIN G, MODICA M, PALEARI S, et al. Assessing disaster risk by integrating natural and socio-economic dimensions: a decision-support tool[J]. Socio-Economic Planning Sciences, 2021, 77: 101032.1-101032.13. [58] GRÜNTHAL G, THIEKEN A H, SCHWARZ J, et al. Comparative risk assessments for the city of cologne—storms, floods, earthquakes[J]. Natural Hazards, 2006, 38(1): 21-44. [59] DI MAURO D, LEPIDI S, DI PERSIO M, et al. Update on monitoring of magnetic and electromagnetic tectonic signals in Central Italy[J]. Annals of Geophysics, 2009, 50(1): 51-60. [60] MARZOCCHI W, NEWHALL C, WOO G. The scientific management of volcanic crises[J]. Journal of Volcanology and Geothermal Research, 2012, 247/248: 181-189. doi: 10.1016/j.jvolgeores.2012.08.016 [61] MIGNAN A, WIEMER S, GIARDINI D. The quantification of low-probability—high-consequences events: part I. a generic multi-risk approach[J]. Natural Hazards, 2014, 73(3): 1999-2022. doi: 10.1007/s11069-014-1178-4 [62] KAPPES M S, GRUBER K, FRIGERIO S, et al. The MultiRISK platform: the technical concept and application of a regional-scale multihazard exposure analysis tool[J]. Geomorphology, 2012, 151/152: 139-155. doi: 10.1016/j.geomorph.2012.01.024 [63] LIU Z Q, NADIM F, GARCIA-ARISTIZABAL A, et al. A three-level framework for multi-risk assessment[J]. Georisk: Assessment and Management of Risk for Engineered Systems and Geohazards, 2015, 9(2): 59-74. doi: 10.1080/17499518.2015.1041989 [64] MARZOCCHI W, GARCIA-ARISTIZABAL A, GASPARINI P, et al. Basic principles of multi-risk assessment: a case study in Italy[J]. Natural Hazards, 2012, 62(2): 551-573. doi: 10.1007/s11069-012-0092-x [65] VLACHOGIANNIS D, SFETSOS A, MARKANTONIS I, et al. Quantifying the occurrence of multi-hazards due to climate change[J]. Applied Sciences, 2022, 12(3): 1218.1-1218.17. [66] MING X D, LIANG Q H, DAWSON R, et al. A quantitative multi-hazard risk assessment framework for compound flooding considering hazard inter-dependencies and interactions[J]. Journal of Hydrology, 2022, 607: 127477.1-127477.16. [67] 李钢,张倪飞,董志骞,等. 多灾害作用下工程结构分析与设计方法研究进展[J]. 土木工程学报,2023,56(8): 9-26.LI Gang, ZHANG Nifei, DONG Zhiqian, et al. Research progress of engineering structure analysis and design methods under muiple harzards[J]. China Civil Engineering Journal, 2023, 56(8): 9-26. [68] 黄朝迎,孙冷. 试论气候异常对重大工程建设的影响[J]. 地理学报,2000,55(增): 5-10.HUANG Chaoying, SUN Leng. Impacts of unusual climate on key project construction[J]. Acta Geographica Sinica, 2000, 55(S): 5-10. [69] 梅恒. 全寿命周期桥梁多灾害概率风险研究[D]. 哈尔滨:哈尔滨工业大学,2019. [70] DENG L, CAI C S. Applications of fiber optic sensors in civil engineering[J]. Structural Engineering and Mechanics, 2007, 25(5): 577-596. doi: 10.12989/sem.2007.25.5.577 [71] XIONG W, CAI C S, KONG X. Instrumentation design for bridge scour monitoring using fiber Bragg grating sensors[J]. Applied Optics, 2012, 51(5): 547-557. doi: 10.1364/AO.51.000547 [72] CHIEW Y M. Scour protection at bridge piers[J]. Journal of Hydraulic Engineering, 1992, 118(9): 1260-1269. doi: 10.1061/(ASCE)0733-9429(1992)118:9(1260) [73] LAUCHLAN C S, MELVILLE B W. Riprap protection at bridge piers[J]. Journal of Hydraulic Engineering, 2001, 127(5): 412-418. doi: 10.1061/(ASCE)0733-9429(2001)127:5(412) [74] ZARRATI A R, GHOLAMI H, MASHAHIR M B. Application of collar to control scouring around rectangular bridge piers[J]. Journal of Hydraulic Research, 2004, 42(1): 97-103. doi: 10.1080/00221686.2004.9641188 [75] WANG K, LIN C P, CHUNG C C. A bundled time domain reflectometry-based sensing cable for monitoring of bridge scour[J]. Structural Control and Health Monitoring, 2019, 26(5): e2345.1-e2345.14. [76] YU J D, LEE J S, YOON H K. Circular time-domain reflectometry system for monitoring bridge scour depth[J]. Marine Georesources & Geotechnology, 2020, 38(1): 312-321. [77] FUNDERBURK M L, TRAN J, TODD M D, et al. Active scour monitoring using ultrasonic time domain reflectometry of buried slender sensors[J]. Smart Material Structures, 2022, 31(1): 015045.1-015045.14. [78] KONG X, CAI C S, HU J X, et al. Field application of an innovative bridge scour monitoring system with fiber Bragg grating sensors[J]. Journal of Aerospace Engineering, 2017, 30(2): B4016008.1-B4016008.14. [79] 向琪芪,李亚东,魏凯,等. 桥梁基础冲刷研究综述[J]. 西南交通大学学报,2019,54(2): 235-248. doi: 10.3969/j.issn.0258-2724.20170373XIANG Qiqi, LI Yadong, WEI Kai, et al. Review of bridge foundation scour[J]. Journal of Southwest Jiaotong University, 2019, 54(2): 235-248. doi: 10.3969/j.issn.0258-2724.20170373 [80] JONGMAN B, WARD P J, AERTS J C J H. Global exposure to river and coastal flooding: long term trends and changes[J]. Global Environmental Change, 2012, 22(4): 823-835. doi: 10.1016/j.gloenvcha.2012.07.004 [81] ALFIERI L, BISSELINK B, DOTTORI F, et al. Global projections of river flood risk in a warmer world[J]. Earth’s Future, 2017, 5(2): 171-182. doi: 10.1002/2016EF000485 [82] WILLNER S N, LEVERMANN A, ZHAO F, et al. Adaptation required to preserve future high-end river flood risk at present levels[J]. Science Advances, 2018, 4(1): eaao1914.1-eaao1914.8. [83] 王唐修,姜鑫民. 谈气候变化影响及对策[J]. 中国能源,2002,156(12): 38-40.WANG Tangxiu, JIANG Xinmin. Influence and strategy of climate change[J]. Energy of China, 2002, 156(12): 38-40. [84] ALIPOUR A, SHAFEI B, SHINOZUKA M. Reliability-based calibration of load and resistance factors for design of RC bridges under multiple extreme events: scour and earthquake[J]. Journal of Bridge Engineering, 2013, 18(5): 362-371. doi: 10.1061/(ASCE)BE.1943-5592.0000369 [85] 孙得璋,陈斌,孙柏涛,等. 桥梁多灾害研究综述[J]. 自然灾害学报,2014,23(6): 32-37.SUN Dezhang, CHEN Bin, SUN Baitao, et al. A summary review of multiple hazards study on bridges[J]. Journal of Natural Disasters, 2014, 23(6): 32-37. [86] 陈梦成,温清清,罗睿,等. 地铁工程钢筋混凝土梁疲劳损伤演化和寿命预测模型研究[J]. 铁道学报,2021,43(1): 160-168.CHEN Mengcheng, WEN Qingqing, LUO Ru, et al. Investigation on prediction models of damage evolution and fatigue lifetime for reinforced concrete beams of metros[J]. Journal of the China Railway Society, 2021, 43(1): 160-168. [87] SPENCER P C, HENDY C R, PETTY R. Quantification of sustainability principles in bridge projects[J]. Proceedings of the Institution of Civil Engineers—Bridge Engineering, 2012, 165(2): 81-89. doi: 10.1680/bren.10.00037 [88] YANG S C, LIU T J, HONG H P. Reliability of tower and tower-line systems under spatiotemporally varying wind or earthquake loads[J]. Journal of Structural Engineering, 2017, 143(10): 04017137.1-04017137.13. [89] DONG Y, FRANGOPOL D M, SAYDAM D. Time-variant sustainability assessment of seismically vulnerable bridges subjected to multiple hazards[J]. Earthquake Engineering & Structural Dynamics, 2013, 42(10): 1451-1467. [90] VAN DE LINDT J W, DAO T N. Performance-based wind engineering for wood-frame buildings[J]. Journal of Structural Engineering, 2009, 135(2): 169-177. doi: 10.1061/(ASCE)0733-9445(2009)135:2(169) [91] CIAMPOLI M, PETRINI F, AUGUSTI G. Performance-based wind engineering: towards a general procedure[J]. Structural Safety, 2011, 33(6): 367-378. doi: 10.1016/j.strusafe.2011.07.001 [92] DAO T N, VAN DE LINDT J W. Loss analysis for wood frame buildings during hurricanes. I: structure and hazard modeling[J]. Journal of Performance of Constructed Facilities, 2012, 26(6): 729-738. doi: 10.1061/(ASCE)CF.1943-5509.0000269 [93] BANERJEE S, GANESH PRASAD G. Seismic risk assessment of reinforced concrete bridges in flood-prone regions[J]. Structure and Infrastructure Engineering, 2013, 9(9): 952-968. doi: 10.1080/15732479.2011.649292 [94] 顾祥林,余倩倩,姜超,等. 城市土木工程基础设施韧性提升理论与方法[J]. 工程力学,2023,40(3): 1-13.GU Xianglin, YU Qianqian, JIANG Chao, et al. Theory and method of resilience enhancement of urban civil engineering infrastructures[J]. Engineering Mechanics, 2023, 40(3): 1-13. [95] KUMAR R, GARDONI P. Effect of seismic degradation on the fragility of reinforced concrete bridges[J]. Engineering Structures, 2014, 79: 267-275. doi: 10.1016/j.engstruct.2014.08.019 [96] KABIR S, PATIDAR S, XIA X L, et al. A deep convolutional neural network model for rapid prediction of fluvial flood inundation[J]. Journal of Hydrology, 2020, 590: 125481.1-125481.45. [97] 董胜,周冲,陶山山,等. 基于ClaytonCopula函数的二维Gumbel模型及其在海洋平台设计中的应用[J]. 中国海洋大学学报(自然科学版),2011,41(10): 117-120.DONG Sheng, ZHOU Chong, TAO Shanshan, et al. Bivariate gumbel distribution based on clayton copula and its application in offshore platform design[J]. Periodical of Ocean University of China, 2011, 41(10): 117-120. [98] LI H N, ZHENG X W, LI C. Copula-based approach to construct a joint probabilistic model of earthquakes and strong winds[J]. International Journal of Structural Stability and Dynamics, 2019, 19(4): 1950046.1-1950046.21. [99] ZHENG X W, LI H N, YANG Y B, et al. Damage risk assessment of a high-rise building against multihazard of earthquake and strong wind with recorded data[J]. Engineering Structures, 2019, 200: 109697.1-109697.14. [100] 陈子燊. 波高与风速联合概率分布研究[J]. 海洋通报,2011,30(2): 159-164.CHEN Zishen. Study on joint probability distribution of wave height and wind velocity[J]. Marine Science Bulletin, 2011, 30(2): 159-164. [101] LI H N, ZHENG X W, LI C. Copula-based joint distribution analysis of wind speed and direction[J]. Journal of Engineering Mechanics, 2019, 145(5): 04019024.1-04019024.12. [102] 杨延凯,马如进,陈艾荣. 基于风险的桥梁多灾害下合理冲刷深度研究[J]. 华南理工大学学报(自然科学版),2016,44(3): 103-109,127.YANG Yankai, MA Rujin, CHEN Airong. Risk-based probe into appropriate scour depth of bridge under multiple hazards[J]. Journal of South China University of Technology (Natural Science Edition), 2016, 44(3): 103-109,127. [103] 谷音,范立础,叶建仁. 基于结构易损性的斜拉桥多灾害安全性能研究[J]. 福州大学学报(自然科学版),2010,38(3): 401-407.GU Yin, FAN Lichu, YE Jianren. The assessment method of safety performance of cable-stayed bridge under multi-hazard based on structure vulnerability[J]. Journal of Fuzhou University (Natural Science Edition), 2010, 38(3): 401-407. [104] 陈力波,王嘉嘉,上官萍. 公路斜交梁桥地震易损性模型研究[J]. 工程力学,2018,35(1): 160-171,181.CHEN Libo, WANG Jiajia, SHANGGUAN Ping. Research of seismic vulnerability model for skew highway girder bridge[J]. Engineering Mechanics, 2018, 35(1): 160-171,181. [105] 郭悬,张琛,王云磊. 浅基础桥梁在锈蚀和主震-余震序列作用下的易损性[J]. 扬州大学学报(自然科学版),2018,21(4): 79-82.GUO Xuan, ZHANG Chen, WANG Yunlei. Seismic fragility analysis of shallow foundation supported bridge under corrosion and mainshock-aftershock sequences[J]. Journal of Yangzhou University (Natural Science Edition), 2018, 21(4): 79-82. [106] 贾布裕,余晓琳,颜全胜. 基于离散动态贝叶斯网络的桥梁状态评估方法[J]. 桥梁建设,2016,46(3): 74-79.JIA Buyu, YU Xiaolin, YAN Quansheng. Method of bridge condition assessment based on discrete dynamic Bayesian networks[J]. Bridge Construction, 2016, 46(3): 74-79. [107] 卢鑫月,许成顺,侯本伟,等. 基于动态贝叶斯网络的地铁隧道施工风险评估[J]. 岩土工程学报,2022,44(3): 492-501.LU Xinyue, XU Chengshun, HOU Benwei, et al. Risk assessment of metro construction based on dynamic Bayesian network[J]. Chinese Journal of Geotechnical Engineering, 2022, 44(3): 492-501. [108] SHAN J Z, ZHUANG C H, LOONG C N. Parametric identification of Timoshenko-beam model for shear-wall structures using monitoring data[J]. Mechanical Systems and Signal Processing, 2023, 189: 110100.1-110100.19. [109] 张望欣,韩强,温佳年,等. 基于地震灾害管理的桥梁网络韧性决策框架[J]. 土木工程学报,2023,56(4): 72-82.ZHANG Wangxin, HAN Qiang, WEN Jianian, et al. A decision framework for improving bridge network resilience based on earthquake disaster management[J]. China Civil Engineering Journal, 2023, 56(4): 72-82. [110] 毛新华,王建伟,袁长伟,等. 基于韧性最优的灾后公路网修复调度研究[J]. 中国公路学报,2022,35(6): 289-298.MAO Xinhua, WANG Jianwei, YUAN Changwei, et al. Restoration scheduling for post-disaster road networks based on resilience optimization[J]. China Journal of Highway and Transport, 2022, 35(6): 289-298. [111] 黄晓明,赵润民. 道路交通基础设施韧性研究现状及展望[J]. 吉林大学学报(工学版),2023,53(6): 1529-1549.HUANG Xiaoming, ZHAO Runming. Status and prospects of highway transportation infrastructure resilience research[J]. Journal of Jilin University (Engineering and Technology Edition), 2023, 53(6): 1529-1549. [112] SHAN J Z, WANG L J, LOONG C N, et al. Rapid seismic performance evaluation of existing frame structures using equivalent SDOF modeling and prior dynamic testing[J]. Journal of Civil Structural Health Monitoring, 2023, 13(2): 749-766. [113] 梁逸文,陈清军. 不同场地条件下远场地震动强度指标与结构最大响应的相关性分析[J]. 力学季刊,2022,43(3): 592-602.LIANG Yiwen, CHEN Qingjun. Correlation analysis between intensity measures of far-field ground motion and maximum structural seismic responses under different site conditions[J]. Chinese Quarterly of Mechanics, 2022, 43(3): 592-602. [114] 宫凤强,李嘉维. 基于PCA-DDA原理的砂土液化预测模型及应用[J]. 岩土力学,2016,37(增1): 448-454.GONG Fengqiang, LI Jiawei. Discrimination model of sandy soil liquefaction based on PCA-DDA principle and its application[J]. Rock and Soil Mechanics, 2016, 37(S1): 448-454. [115] 季家威. PCA在环境影响下结构损伤识别中的应用[D]. 苏州:苏州科技大学,2019. [116] 李岩,张久鹏,陈子璇,等. 基于PCA-PSO-SVM的沥青路面使用性能评价[J]. 吉林大学学报(工学版),2023,53(6): 1729-1735.LI Yan, ZHANG Jiupeng, CHEN Zixuan, et al. Evaluation of asphalt pavement performance based on PCA-PSO-SVM[J]. Journal of Jilin University (Engineering and Technology Edition), 2023, 53(6): 1729-1735. [117] 李宏男,张文圣,付兴. 基于大数据深度学习的输电塔结构抗风易损性评估[J]. 土木工程学报,2022,55(9): 54-64.LI Hongnan, ZHANG Wensheng, FU Xing. Fragility assessment of a transmission tower subjected to wind load based on big data and deep learning[J]. China Civil Engineering Journal, 2022, 55(9): 54-64. [118] 马世龙,乌尼日其其格,李小平. 大数据与深度学习综述[J]. 智能系统学报,2016,11(6): 728-742.MA Shilong, WUNIRI Qiqige, LI Xiaoping. Deep learning with big data: state of the art and development[J]. CAAI Transactions on Intelligent Systems, 2016, 11(6): 728-742. [119] 吕五一,刘仍奎,张秋艳,等. 基于集成学习算法的轨道几何状态短期预测模型[J]. 铁道建筑,2021,61(4): 107-110,115.LYU Wuyi,LIU Rengkui,ZHANG Qiuyan, et al. Short-term prediction model of track geometry state based on ensemble learning algorithm[J]. Railway Engineering, 2021, 61(4): 107-110,115. [120] 杜宪亭,夏禾,李慧乐,等. 基于改进高斯精细积分法的车桥耦合振动分析框架[J]. 工程力学,2013,30(9): 171-176.DU Xianting, XIA He, LI Huile, et al. Dynamic analysis framework of train-bridge system based on improved gauss precise integration method[J]. Engineering Mechanics, 2013, 30(9): 171-176. -
下载:
下载:
点击查看大图
计量
- 文章访问数: 374
- HTML全文浏览量: 112
- PDF下载量: 96
- 被引次数: 0