Influence Analysis of Vibration Control Parameters for High-Speed Maglev Train-Bridge Coupling
-
摘要:
磁浮列车悬浮系统控制参数取值不当可能导致车-桥系统异常振动. 因此,明确悬浮系统控制参数与磁浮车-桥系统动力响应之间的关系十分重要. 首先,建立包含比例-微分控制的5节编组磁浮列车动力学模型和20跨简支梁桥有限元模型;其次,与实测结果进行对比验证所建立模型的正确性;最后,计算车速430 km/h时不同控制参数下列车和桥梁的动力响应. 结果表明:增大比例系数会使悬浮和导向系统刚度增大,增大微分系数会使悬浮和导向系统阻尼增大;车体竖向加速度随比例和微分系数的增大而增大,车体横向加速度随比例系数的增大而增大;悬浮间隙和桥梁竖向加速度均随比例系数的增大而减小,随微分系数的增大而增大;导向间隙随微分系数的增大而减小,比例系数对导向间隙的影响较小;桥梁横向加速度随比例系数的增大而减小,随微分系数的增大而增大;桥梁竖向加速度主要受电磁力中悬浮电磁铁长度特征频率1倍~12倍频的影响,桥梁横向加速度主要受导向磁极长度特征频率及其2倍频和导向电磁铁长度特征频率2倍频及4倍频的影响;为减小车-桥系统动力响应,综合建议竖向比例和微分系数的取值范围分别为3000~4000和10~25,横向比例和微分系数的取值范围分别为4000~5000和10~25.
Abstract:Improper control parameters of the suspension system of maglev trains may lead to abnormal vibration of the train-bridge system. Therefore, it is important to clarify the relationship between the control parameters of the suspension system and the dynamic response of the maglev train-bridge system. Firstly, the dynamic model of a 5-car maglev train with proportional-differential control, as well as the finite element model of a 20-span simply supported beam bridge was established. Secondly, the correctness of the models was verified by comparing them with the measured results. Finally, the dynamic responses of the train and bridge under different control parameters at 430 km/h were calculated. The results show that increasing the proportional coefficient will increase the stiffness of the suspension and guidance system, and increasing the differential coefficient will increase the damping of the suspension and guidance system. The vertical acceleration of the car body increases with the increase in the proportional and differential coefficients, and the lateral acceleration of the car body increases with the increase in the proportional coefficient. The suspension gap and the vertical acceleration of the bridge decrease with the increase in the proportional coefficient, and they increase with the increase in the differential coefficient. The guidance gap decreases with the increase in the differential coefficient, and the proportional coefficient has little effect on the guidance gap. The lateral acceleration of the bridge decreases with the increase in the proportional coefficient and increases with the increase in the differential coefficient. The vertical acceleration of the bridge is mainly affected by a characteristic frequency of 1–12 times of the length of the suspended electromagnet in the electromagnetic force, and the lateral acceleration of the bridge is mainly affected by the characteristic frequency and frequency of 2 times of the length of the guidance pole, as well as the characteristic frequency of 2 times and 4 times of the length of the guidance electromagnet. In order to reduce the dynamic response of the train-bridge system, it is suggested that the values of vertical proportional and differential coefficients should be 3 000–4 000 and 10–25, respectively, and the values of lateral proportional and differential coefficients should be 4 000–5 000 and 10–25, respectively.
-
磁浮列车是一种新型交通工具,具有非接触式支撑、低噪音和爬坡能力强等优点[1-2],近年来在国内外得到快速的发展与应用[3]. 常导磁浮列车的悬浮系统是一个先天开环不稳定的系统,需要引入控制器对其进行控制[4-5]. 然而,调试悬浮系统控制参数需要大量的时间,且往往都依赖于专家经验,当控制参数取值不恰当时可能引发列车和轨道异常振动[6-7],造成乘车舒适性差、运行平稳性差和噪音高等问题. 因此,明确控制参数与磁浮车-桥耦合系统动力响应之间的关系,选择合适的控制参数对磁浮列车的发展具有重要意义.
针对磁浮列车-桥梁耦合系统悬浮控制参数的分析,目前已有学者进行了相关研究:韩霄翰等[8]基于单点悬浮控制系统模型,对控制参数引起的车轨耦合失稳振动的特性进行了分析并发现,当比例系数取值处于52~55时,悬浮模块会发生15~25 Hz的周期振动,比例系数取值大于55时,悬浮模块振动发散直至与道岔梁吸死; Feng等[9]分析了延时影响下运行速度和控制参数对系统动态响应的影响规律;赵春发等[10]以单电磁铁为对象,进行了控制参数的影响分析,发现车体二系悬挂频率、轨道基频和控制系统特征频率任意两者互相接近时容易发生车轨共振;蔡文涛等[11]讨论了单电磁铁控制器在不同控制参数下,悬浮间隙和加速度的变化曲线,表明加速度和位移反馈系数的增大,使得悬浮系统的加速度增大,其中位移反馈系数会使响应时间减少,速度反馈系数的增大,会使系统阻尼增大,稳定更快;梁鑫等[12]建立单磁铁-轨道-悬浮系统模型,研究了悬浮控制系统反馈系数对磁浮列车稳定性的影响. 以上研究均以单电磁铁作为研究对象,并没有建立完整的车-桥耦合系统模型,且没有给出具体的控制参数范围.
陆海英等[6]分析了单级悬浮控制与双级悬浮控制的效果差异,并表明中低速磁浮列车在弹性轨道梁上起浮时,容易发生悬浮失稳的异常振动现象; 汤港归等[13]探究了PD (比例-微分)控制参数在磁浮车辆起浮过程中对起浮稳定性与振动舒适性的影响,指出比例控制参数有上下限值. 以上2项研究主要针对磁浮列车起浮过程进行研究,并没有分析列车运行过程中控制参数的影响. Sun等[14]采用 Hopf 分岔准则对磁悬浮列车-轨道耦合振动进行了研究,分析了控制参数对耦合振动的影响规律和系统稳定范围,然而该研究仅仅是二维模型,且只考虑了桥梁前2阶模态;杨志南等[15]仿真分析了不同悬浮控制参数条件下磁浮列车以 30 km/h 通过道岔梁时的动力学响应,并建议间隙反馈系数为 7000~8000 ,速度反馈系数为 45~60,该研究针对道岔,且车速较低; Xiang等[16-17]基于 PD 控制器,建立了包括三维磁悬浮列车、导轨、桥墩和电磁力的空间耦合振动模型,分析了控制回路失效时列车和轨道动力响应变化; 谢云德等[18]仿真分析了弹性轨道阻尼、加速度反馈增益等对静态悬浮稳定性和动态特性的影响,该研究仅为2自由度模型,且只考虑了铅锤方向的系统特性. 综上可知,目前通过建立较为完整的高速磁浮车-桥空间耦合系统模型来研究控制参数对车-桥动力响应影响的研究较少.
为明确悬浮系统控制参数与高速磁浮车-桥耦合系统动力响应之间的关系,本文拟建立高速磁浮车-桥空间耦合振动模型;探讨430 km/h车速下竖向、横向控制参数与耦合系统动力响应之间的关系,以期对悬浮系统控制参数的取值提供建议.
1. 磁浮车-桥耦合振动模型
1.1 车辆模型
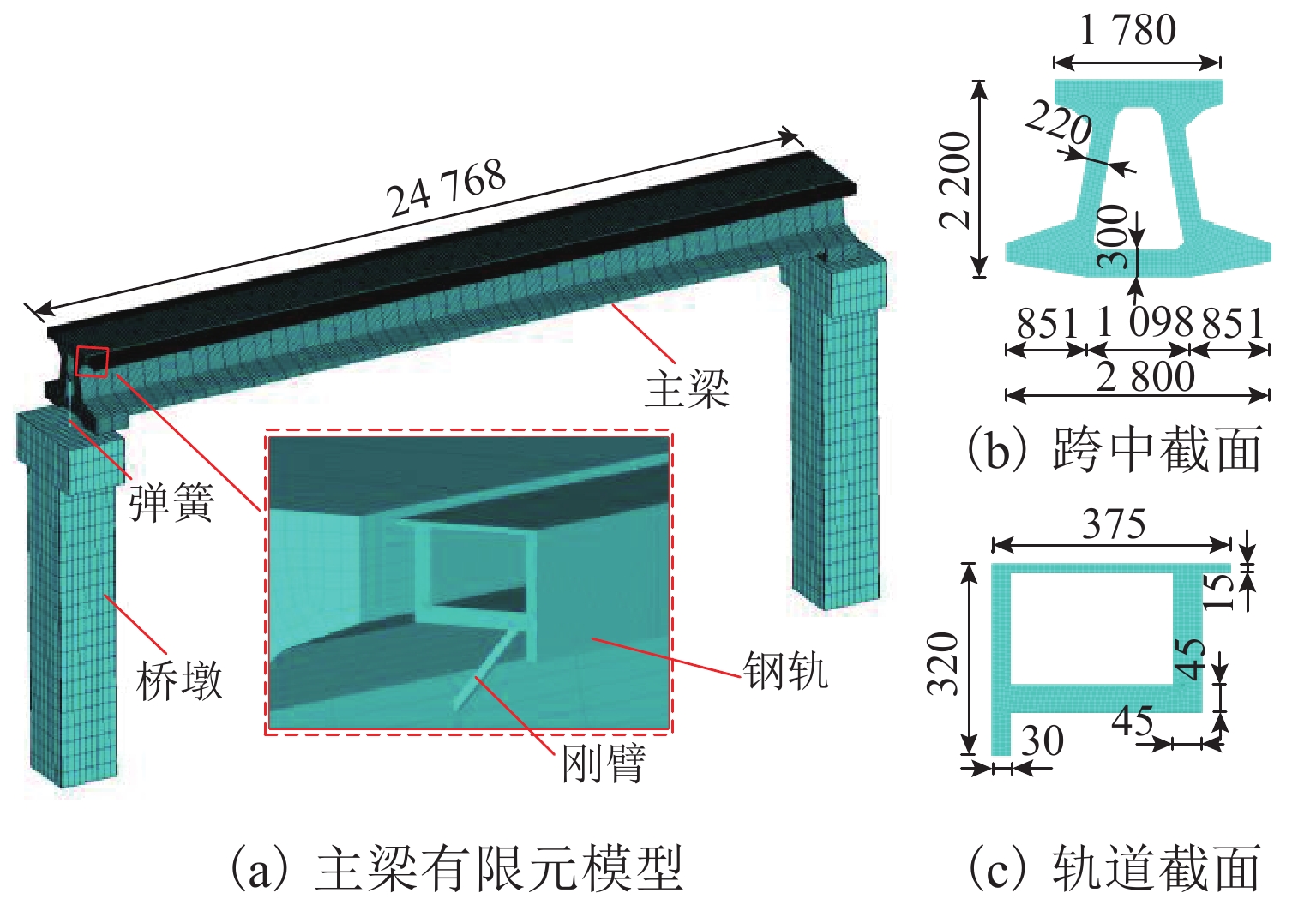
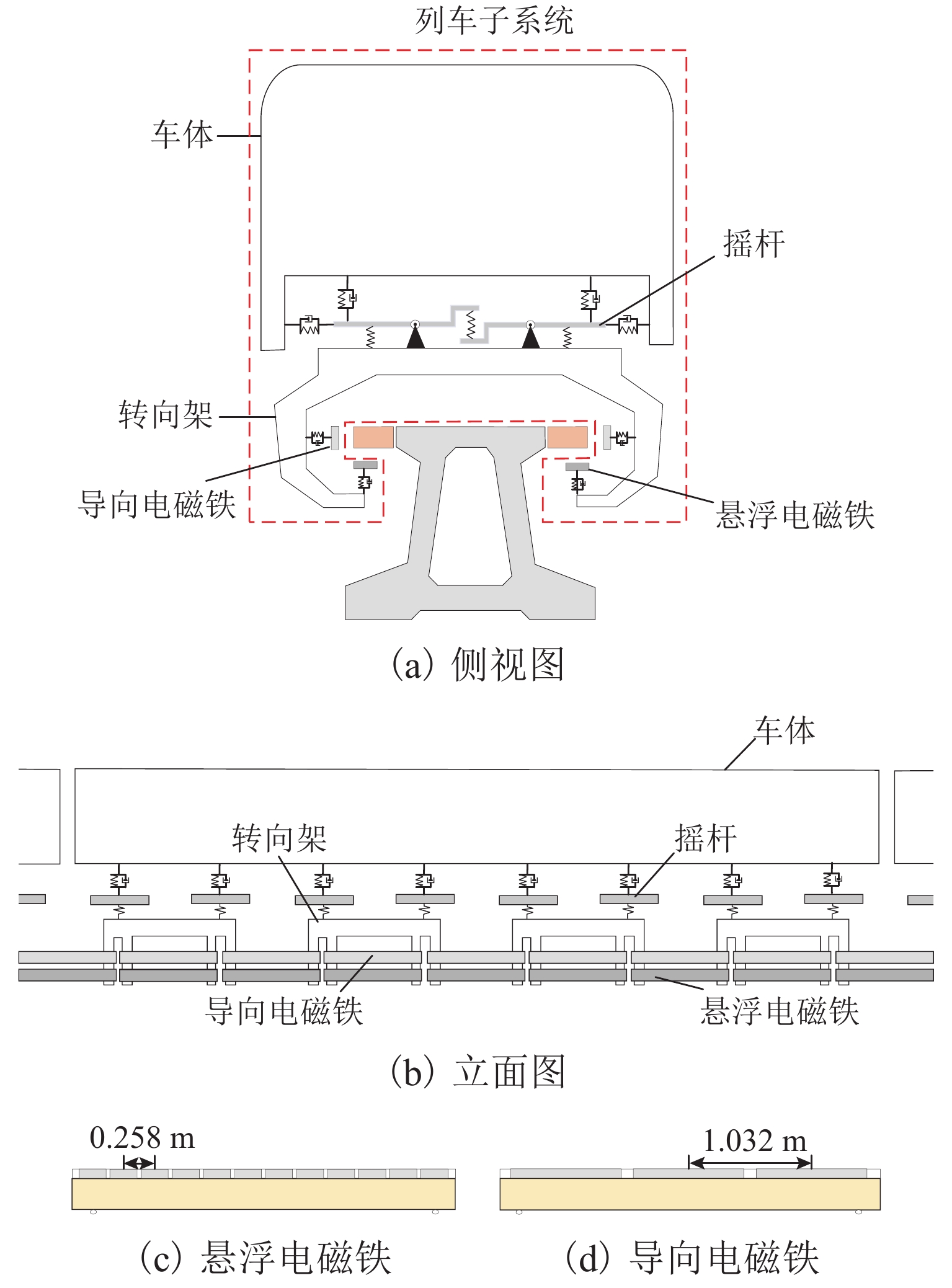
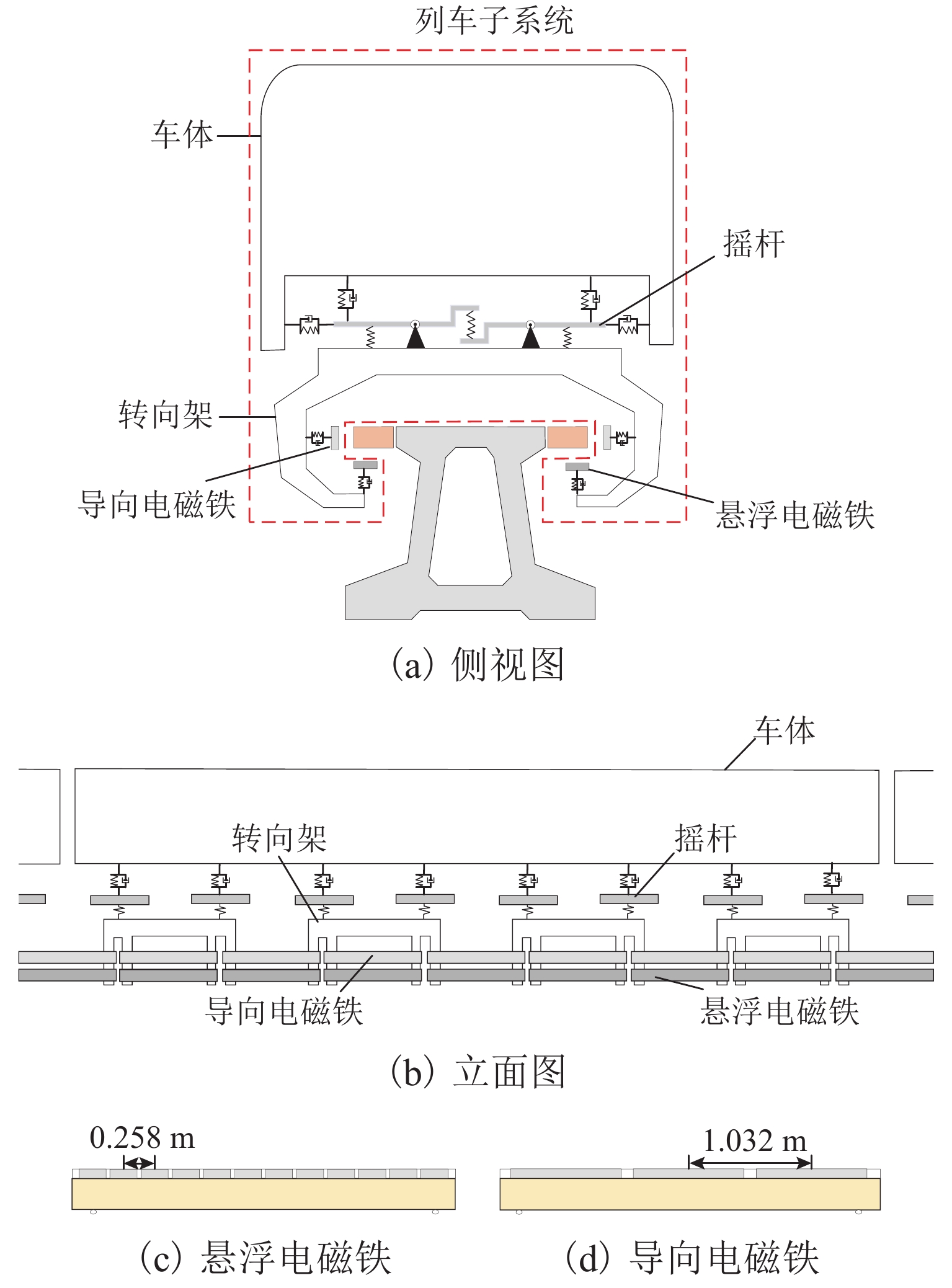
本文以德国TR08型高速磁浮列车为研究对象,将车辆各部分视为刚体,采用多体动力学方法建立车辆模型,如图1 (a)、(b)所示. 磁浮列车由n节相同的车辆组成,每节车辆包含车体、转向架、摇杆、悬浮磁铁和导向磁铁5个部分. 车体与转向架之间通过摇杆、二系弹簧元件连接,转向架与电磁铁之间通过一系弹簧元件连接,相邻两节车首尾转向架之间通过一对悬浮电磁铁和一对导向电磁铁连接. 每个悬浮电磁铁上安装有12个磁极,磁极中心间距为0.258 m,每个导向电磁铁上安装有3个磁极,磁极中心间距为1.032 m,如图1(c)、(d)所示,车辆参数见表1.
表 1 车辆模型参数Table 1. Parameters of train model参数 数值 车体质量/(× 104 kg) 3.90 车体侧滚质量惯性矩/(× 104 kg•m2) 6.46 车体摇头质量惯性矩/(× 104 kg•m2) 1.75 车体点头质量惯性矩/(× 104 kg•m2) 1.76 一系/二系弹簧竖向刚度/(×106 N•m−1) 20/2 一系/二系弹簧竖向阻尼/(×103 N•s•m−1) 5/5 一系/二系弹簧横向刚度/(×106 N•m−1) 28/2 一系/二系弹簧横向阻尼/(×102 N•s•m−1) 5/20 空气弹簧刚度竖向/(× 106 N•m−1) 1.90 在车辆模型中,车体考虑横移、沉浮、侧滚、点头和摇头5个自由度,转向架考虑自身的横移、沉浮、点头和摇头以及2个C型框架的相对转动6个自由度,摇杆只考虑侧滚1个自由度,悬浮磁铁考虑沉浮和点头2个自由度,导向磁铁考虑横移和摇头 2 个自由度. 车辆模型的总自由度数为109n−8,n为列车节数. 基于上述车辆模型,根据达朗贝尔原理可建立其相应的动力方程为
MV¨UV(t)+CV˙UV(t)+KVUV(t)=FVB(t), (1) 式中:t为列车运行时间,${{\boldsymbol{M}}_{\rm{V}}}$、${{\boldsymbol{C}}_{\rm{V}}}$、${{\boldsymbol{K}}_{\rm{V}}}$分别为车辆模型的质量、阻尼和刚度矩阵,$ {{\boldsymbol{U}}_{\mathrm{V}}} $、$ {{{\dot {\boldsymbol{U}}}}_{\mathrm{V}}} $、$ {{{\ddot {\boldsymbol{U}}}}_{\mathrm{V}}} $分别为车辆模型的位移、速度和加速度向量,${{\boldsymbol{F}}_{{\rm{VB}}}}$为作用在车辆模型中的磁轨相互作用力.
车体竖向自振频率为2.17 Hz,横向自振频率为5.73 Hz.
1.2 桥梁模型
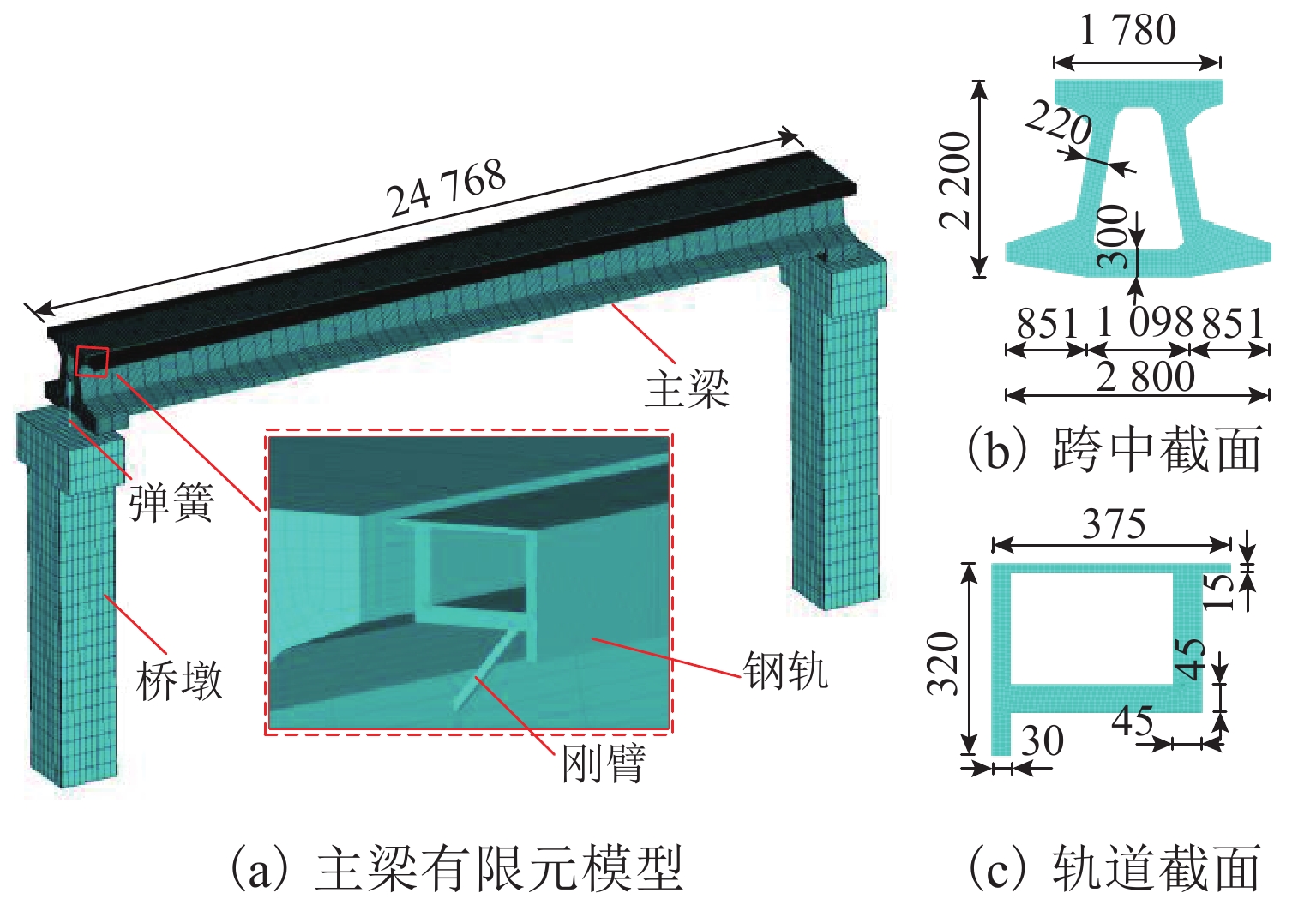
桥梁为单线多跨简支梁桥,标准跨度为24.768 m. 桥梁由混凝土主梁、钢制导轨和混凝土桥墩组成. 钢轨位于主梁上翼缘两侧,通过多对铸铁连接件与主梁连接,桥墩与主梁之间安装支座. 主梁截面梁顶宽1.78 m,梁底宽2.8 m,梁高2.2 m,轨道截面高0.32 m,宽0.375 m[19],桥墩高度为10 m. 采用有限元方法建立桥梁模型,其中,主梁、钢轨和桥墩均采用铁木辛柯梁单元模拟,钢轨与主梁之间采用刚臂连接,主梁与桥墩之间的支座采用线性弹簧阻尼单元模拟. 忽略混凝土桩基与土体之间的相互作用,将桥墩底部设置为固定约束. 基于上述建模方案建立的桥梁有限元模型如图2所示,相关参数见表2.
表 2 桥梁模型参数Table 2. Parameters of bridge model参数 数值 主梁/钢轨/桥墩密度/(kg•m−3) 2551/7850/2500 主梁/钢轨/桥墩弹性模量/GPa 44.5/206.0/30.0 主梁/钢轨/桥墩泊松比 0.2/0.3/0.2 采用直接刚度法建立桥梁动力方程为
MB¨UB(t)+CB˙UB(t)+KBUB(t)=FBV(t), (2) 式中:$ {{{{\boldsymbol{M}}}}_{\mathrm{B}}} $、$ {{{{\boldsymbol{C}}}}_{\mathrm{B}}} $、$ {{{{\boldsymbol{K}}}}_{{{\mathrm{B}}}}} $分别为桥梁模型的质量、阻尼和刚度矩阵,$ {{{{\boldsymbol{U}}}}_{\mathrm{B}}} $、$ {{{\dot {\boldsymbol{U}}}}_{\mathrm{B}}} $、$ {{{\ddot {\boldsymbol{U}}}}_{\mathrm{B}}} $分别为桥梁模型的位移、速度和加速度向量,$ {{\boldsymbol{F}}_{{\mathrm{BV}}}} $为作用在桥梁模型的磁轨相互作用力.
考虑到所述桥梁为混凝土桥,故瑞利阻尼比取2%,主梁前三阶竖向自振频率分别为8.14、28.43、57.06 Hz.
1.3 悬浮控制器模型
磁浮列车是一个多电磁铁悬浮系统,通过解耦[20],磁浮车的悬浮控制可以分解为单个电磁铁的悬浮控制问题[21]. 电磁力的计算主要取决于回路电流和电磁铁与轨道之间的间隙,如式(3).
fe(ie(t),he(t))=μ0N2eAe4(ie(t)he(t))2, (3) 式中:$ {f_e} $为电磁力,$ {i_e} $为所计算磁极位置处线圈的电流值,$ {h_e} $为所计算磁极处的磁浮间隙,μ0为空气磁导率,Ne为磁铁绕组匝数,Ae为磁极面积,e为磁极编号.
磁浮列车处于静平衡状态时的电磁力为
fe(i0,h0)=μ0N2eAe4(i0h0)2=P0, (4) 式中:$ {i_0} $为额定电流;$ {h_0} $为额定间隙;对于悬浮控制,$ {P_0} $为分布在第e个悬浮磁极的磁浮列车重量,对于导向控制,$ {P_0} $为分布在第e个导向磁极的横向预紧力,参考文献[16],本文横向预紧力取3.6 kN/m.
电磁铁在垂直方向上的动力方程为
md2he(t)dt2=mg+fd(t)−fe(ie(t),he(t)), (5) 式中: m为单电磁铁的质量,${f_{\mathrm{d}}}(t)$为施加在电磁铁上的外力.
将式(5)在平衡点($ {i_0} $,$ {h_0} $)附近进行泰勒级数展开(令${f_{\rm{d}}}$=0)并忽略高阶项得到
mΔ¨he=KhΔhe−KiΔie, (6) 式中:${K_{\rm{i}}} = \dfrac{{{\mu _0}{N_e}^2{A_e}{i_0}}}{{2{h_0^2}}}$;${K_{\rm{h}}} = \dfrac{{{\mu _0}{N_e}^2{A_e}{i_0}^2}}{{2{h_0^3}}}$;$ \Delta {h_e} $为悬浮隙变化量,$\Delta {h_e} = {h_e}-{h_0}$;$ \Delta {i_e} $为电流变化量,$\Delta {i_e} = {i_e}-{i_0}$.
本文采用工业上常用的PD控制器进行控制,并且忽略电压和电流之间的延迟,通过直接调节电流达到控制电磁力的目的,即
Δie=KPΔhe+KDΔ˙he, (7) 式中:${K_{\rm{P}}}$和${K_{\rm{D}}}$为比例增益系数和微分增益系数,其取值方法主要参考文献[13],由于悬浮系统和导向系统的初始参数不同,因此,悬浮和导向控制系统的增益系数取值也不相同.
将式(7)代入式(6),可得到平衡点附件闭环悬浮系统动力学方程为
mΔ¨he+KiKDΔ˙he+(KiKP−Kh)Δhe=0. (8) 由式(8)可以得到
{ωs=√KiKP−Khm,ζs=KiKD2√m(KiKP−Kh), (9) 式中:$ {\omega _{\text{s}}} $为闭环悬浮系统的圆频率,$ {\zeta _{\text{s}}} $为闭环悬浮系统的阻尼比.
2. 磁浮车-桥耦合振动求解及模型验证
2.1 磁浮车-桥耦合振动求解
联立式(1)和式(2),可建立磁浮车-桥耦合振动方程,如式(10),本文采用分离迭代法进行求解.
[MV00MB][¨UV¨UB] + [CVCVBCBVCB][˙UV˙UB]+[KVKVBKBVKB][UVUB] = [FVFB], (10) 式中: KBV和KVB分别为由车-桥动力相互作用引起的刚度项,CBV和CVB分别为由车-桥动力相互作用引起的阻尼项,$ {{F}}_{\text{V}}、{{F}}_{\text{B}} $分别为由轨道不平顺引起的车辆模型和桥梁模型的外荷载向量.
2.2 模型验证
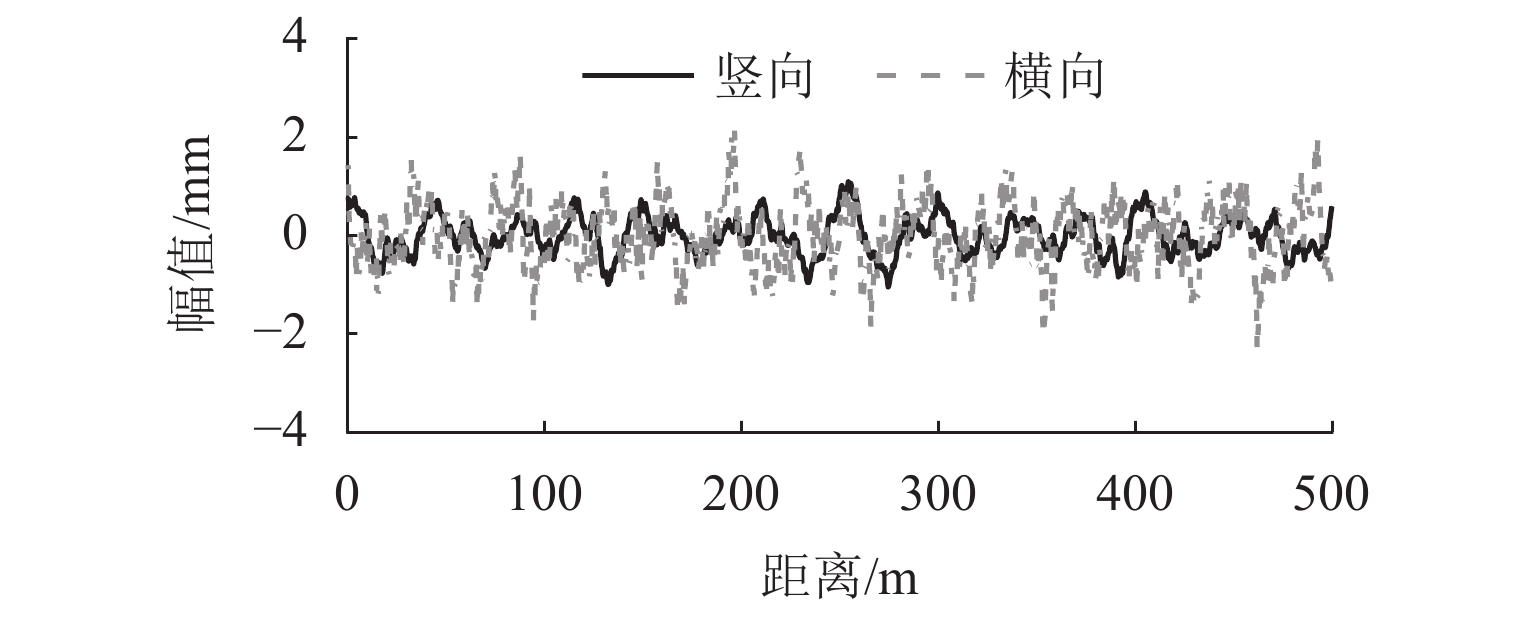
以上海高速磁浮线为背景,通过与实测结果对比验证本文计算模型的正确性. 验证所用磁浮列车采用5节车编组,桥梁跨数考虑20跨. 采用Shi等[22]根据上海轨道不平顺实测结果拟合的七参数PSD函数生成轨道不平顺样本,车速v为 430 km/h,模拟得到的轨道竖向和横向不平顺样本如图3所示. 其中,竖向和横向不平顺幅值分别为1.12 mm和2.31 mm. 采用第2.1节所述方法计算耦合系统动力响应,时间步长取1 × 10−3 s.
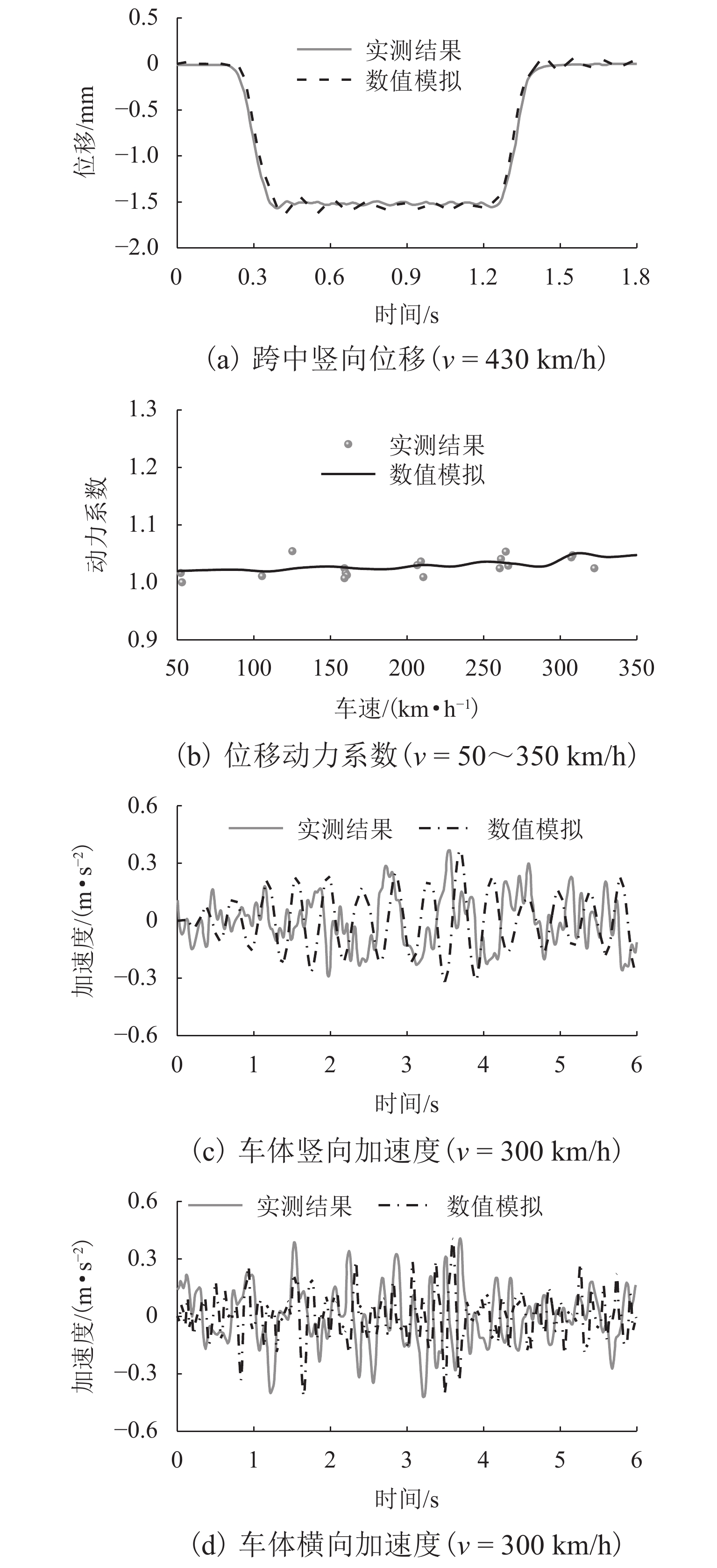
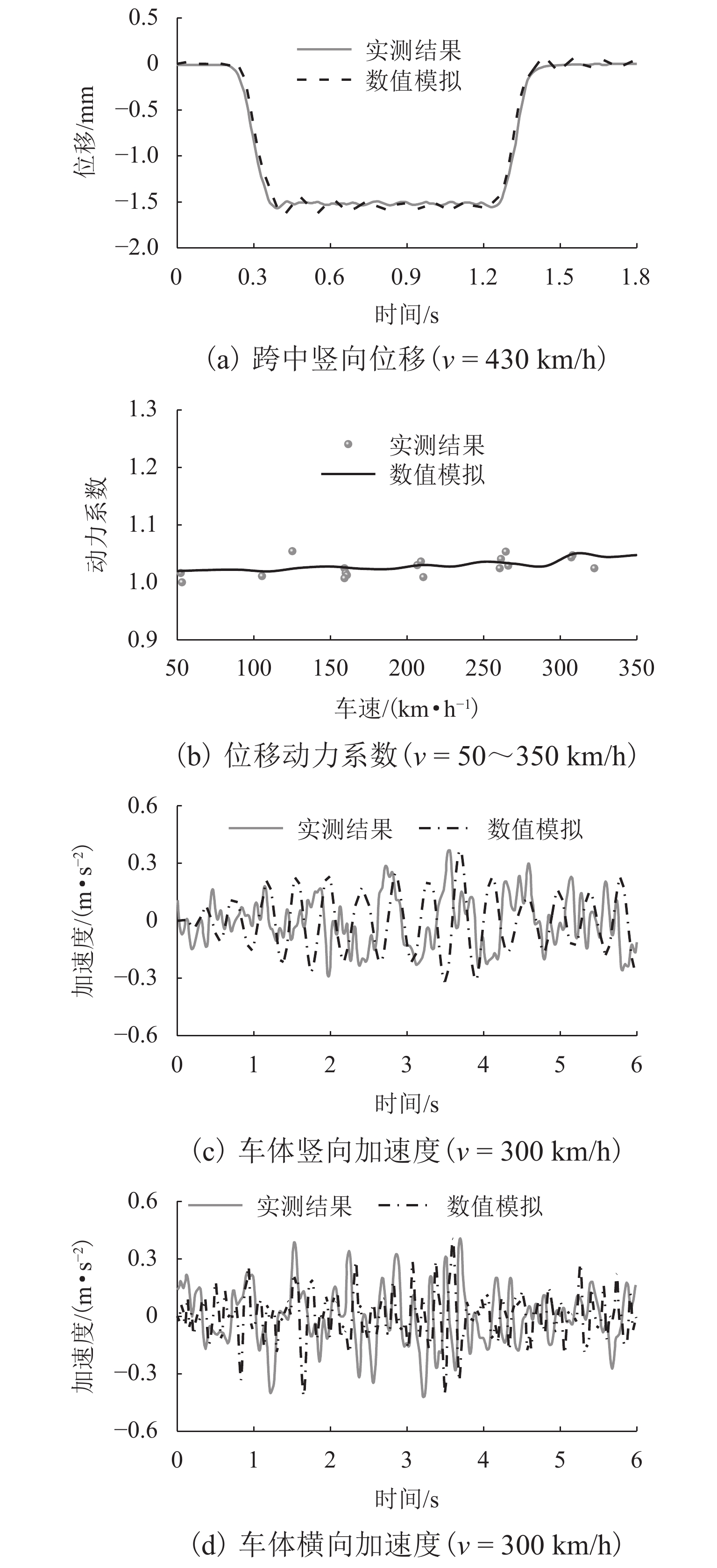
图4 (a) 为第10跨主梁跨中竖向位移计算结果与实测结果的对比,其中实测结果来源于文献[23]. 从图中可以看出:计算结果与实测结果吻合良好,其中前者与后者的幅值分别为1.63 mm和1.57 mm,相对误差为3.82%. 图4 (b) 给出了50~350 km/h车速范围内,第10跨主梁跨中竖向位移动力系数计算结果与实测结果的对比,其中实测结果来源于文献[24]. 从图中可以看出:在计算车速范围内,2种结果的变化趋势基本一致. 为进一步说明本文计算模型的正确性,图4 (c)和图4 (d) 中还给出了车速300 km/h下第2节车车体竖向和横向加速度计算结果与实测结果对比情况,其中车体加速度实测结果来源于文献[25]. 从图中可以看出,车体加速度的数值模拟结果与实测结果的幅值及变化趋势基本一致. 通过以上对比可以说明,本文建立的磁浮车-桥模型具有良好的计算精度.
3. 磁浮车-桥耦合振动控制参数影响分析
本节计算了不同控制参数下5车编组磁浮列车以430 km/h车速通过20跨简支梁桥时车辆和桥梁的动力响应. 以下研究中,竖向控制参数KP1变化范围为2000~15000,KD1变化范围为10~125,横向控制参数KP2变化范围为2000~15000,KD2变化范围为10~125. 车辆动力响应取第2节车为代表,桥梁动力响应取第10跨为代表.
3.1 竖向控制参数
3.1.1 车辆动力响应
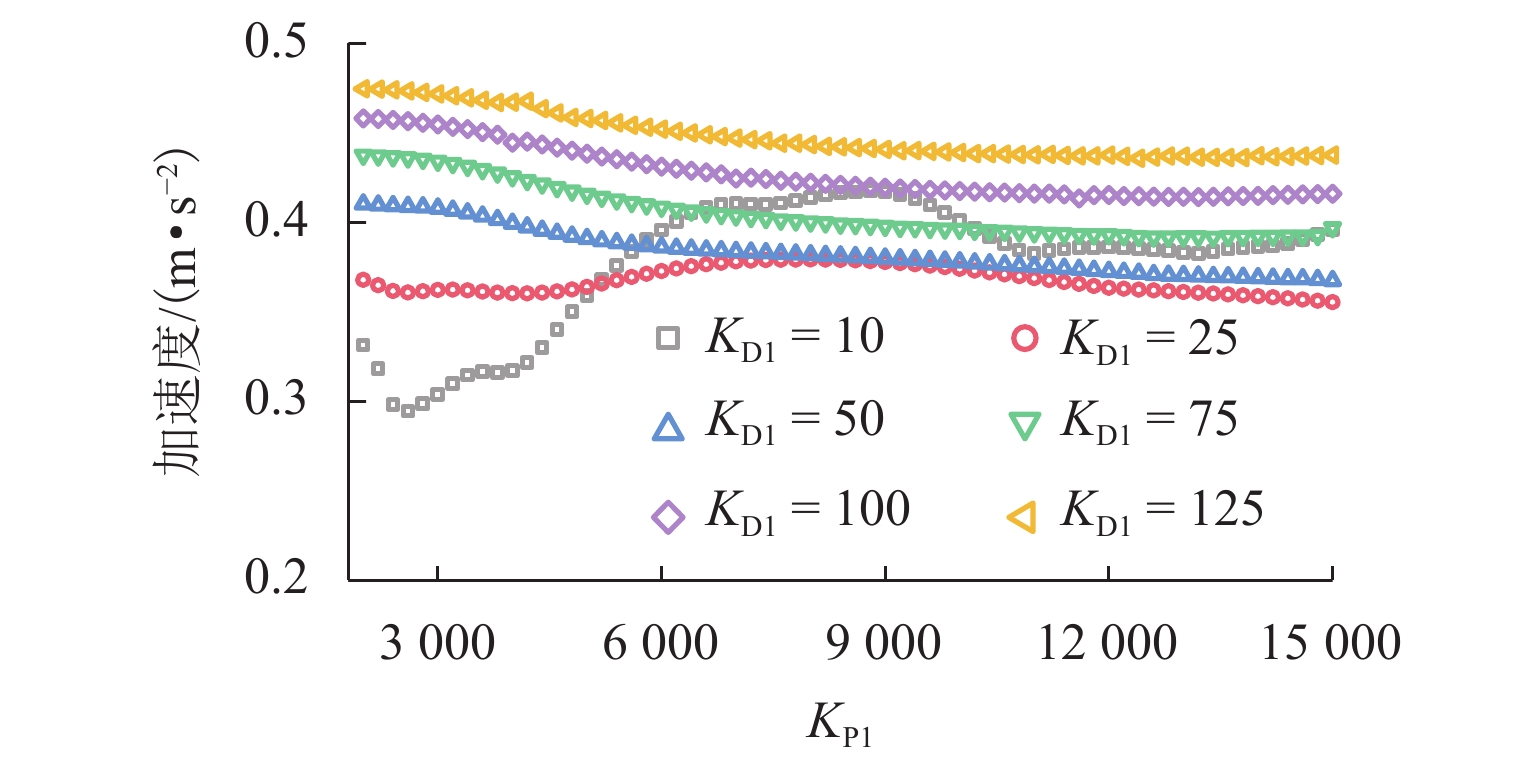
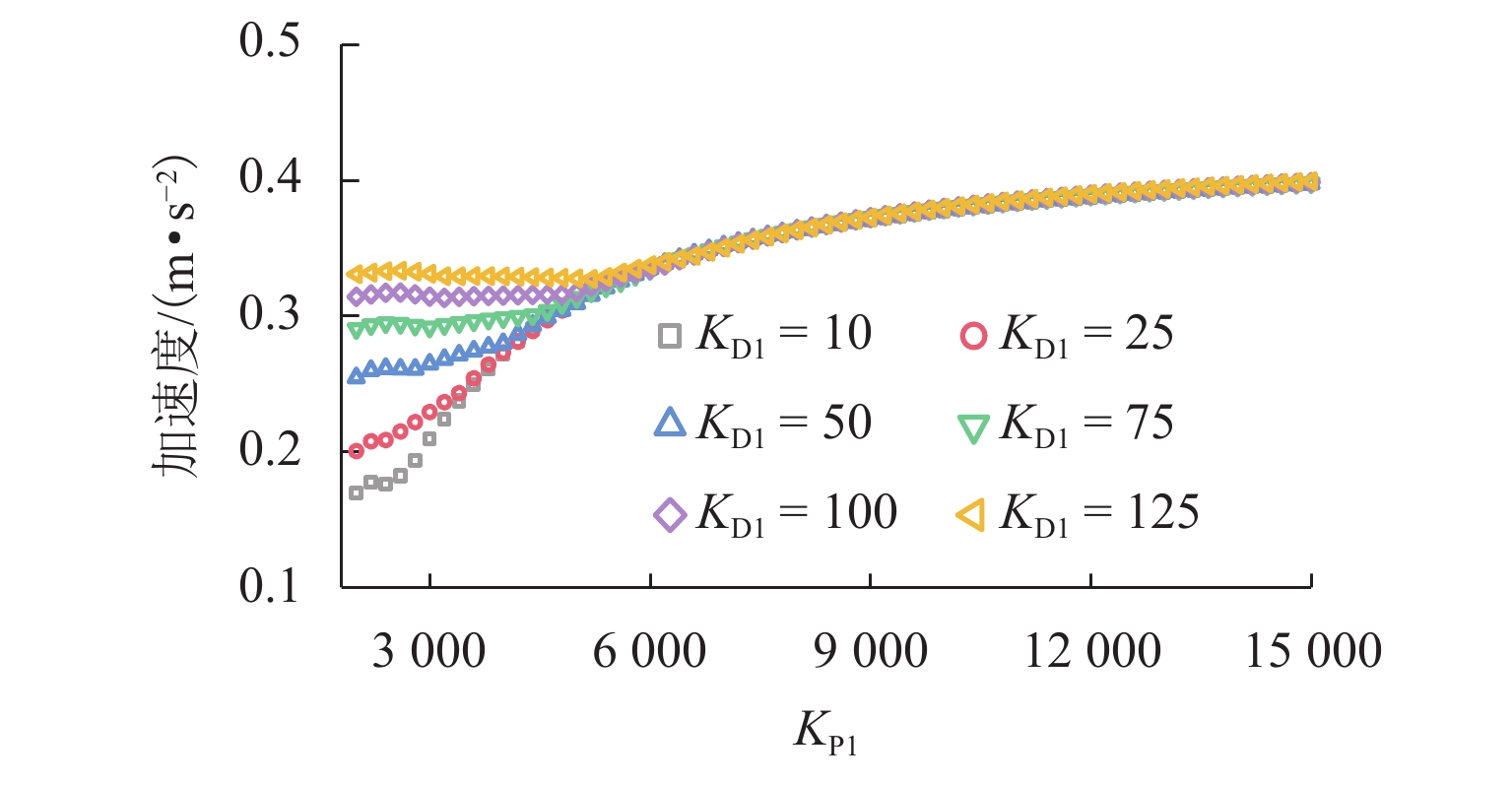
不同控制参数下车体竖向加速度最大值变化情况如图5所示. 从图5中可以看出:当KP1 < 6000时,车体竖向加速度随KP1的增大而增大,且随着KD1的增大,车体竖向加速度受KP1的影响逐渐减小,曲线趋于平缓,同一KP1下,车体竖向加速度随KD1的增大而增大; 当KP1 > 6000时,车体竖向加速度随KP1的增大先增大后逐渐平稳,车体竖向加速度受KD1影响很小.
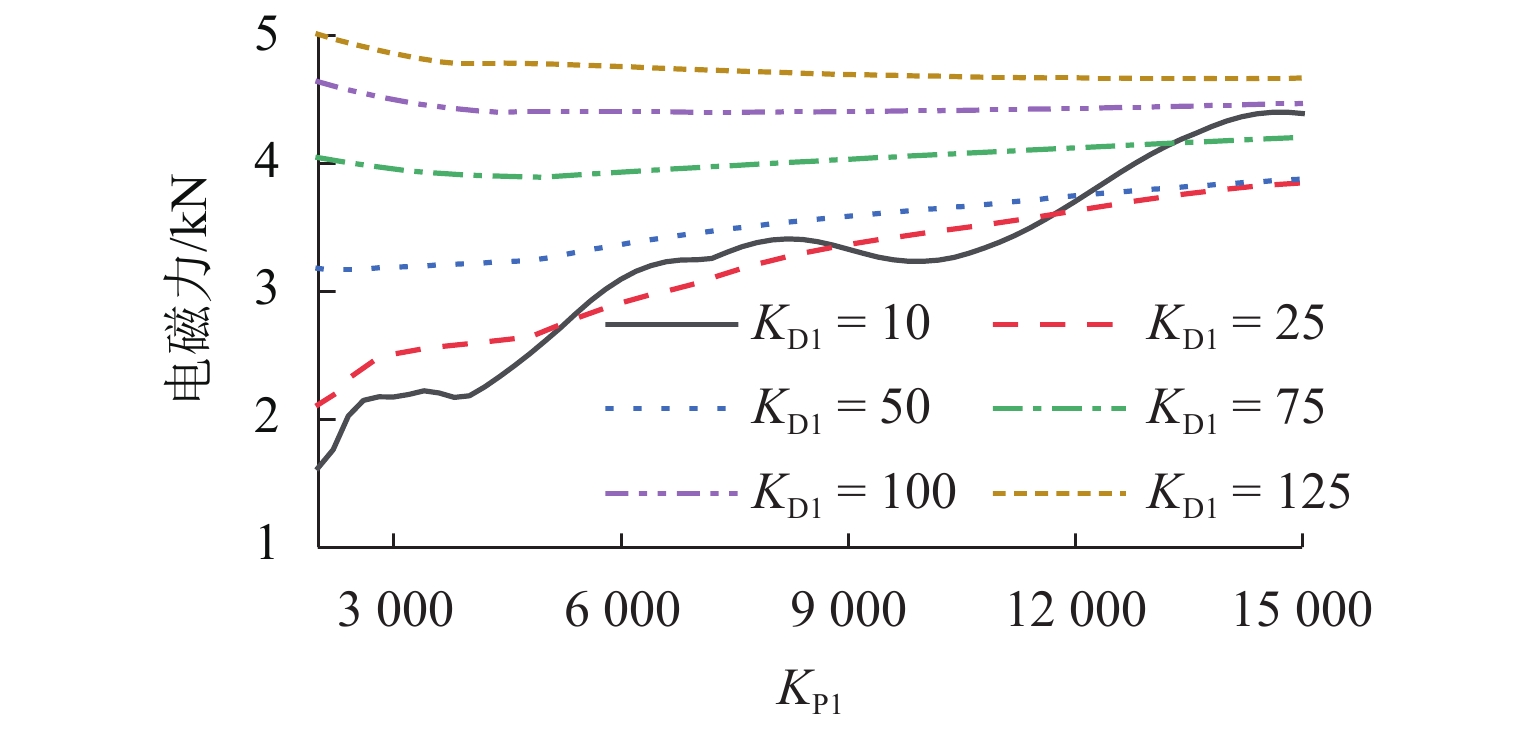
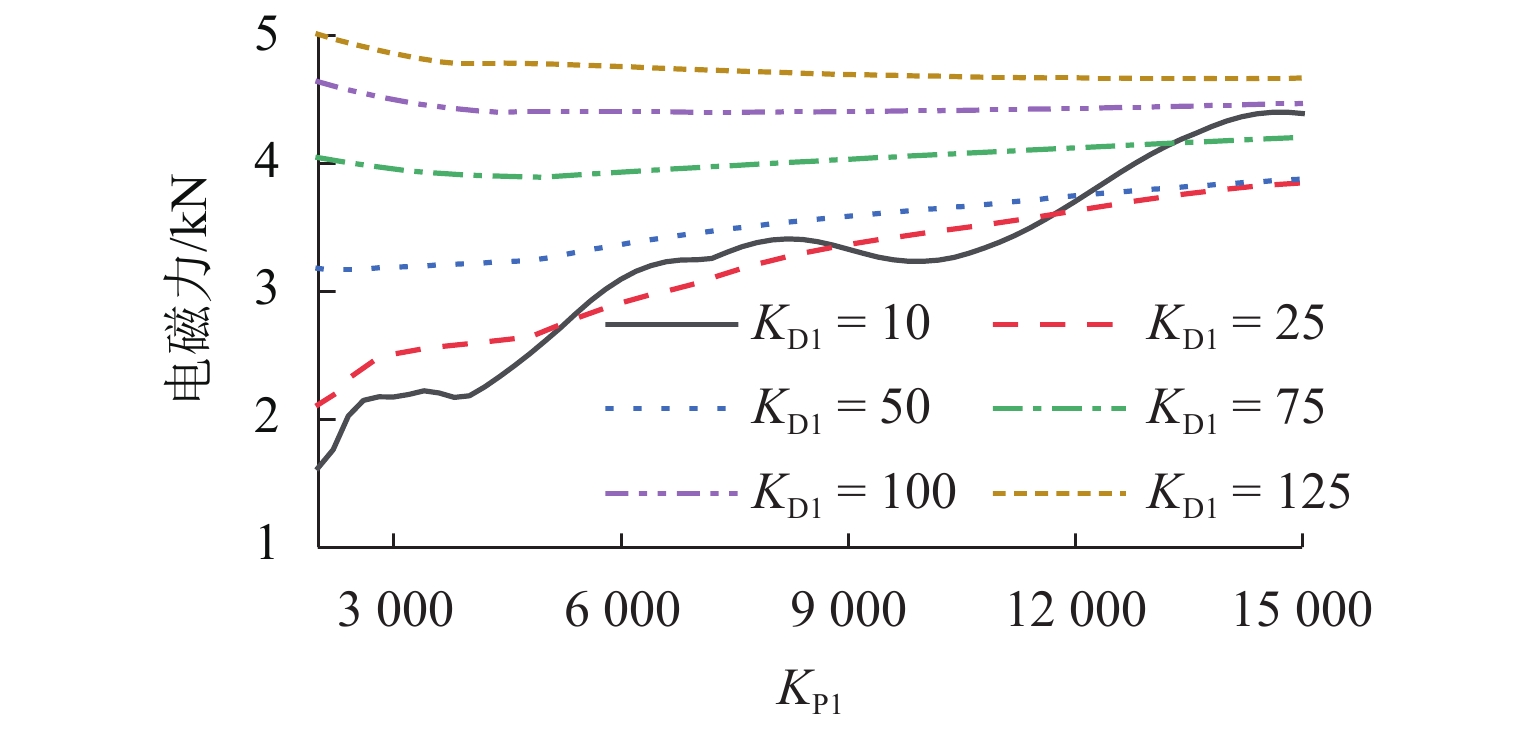
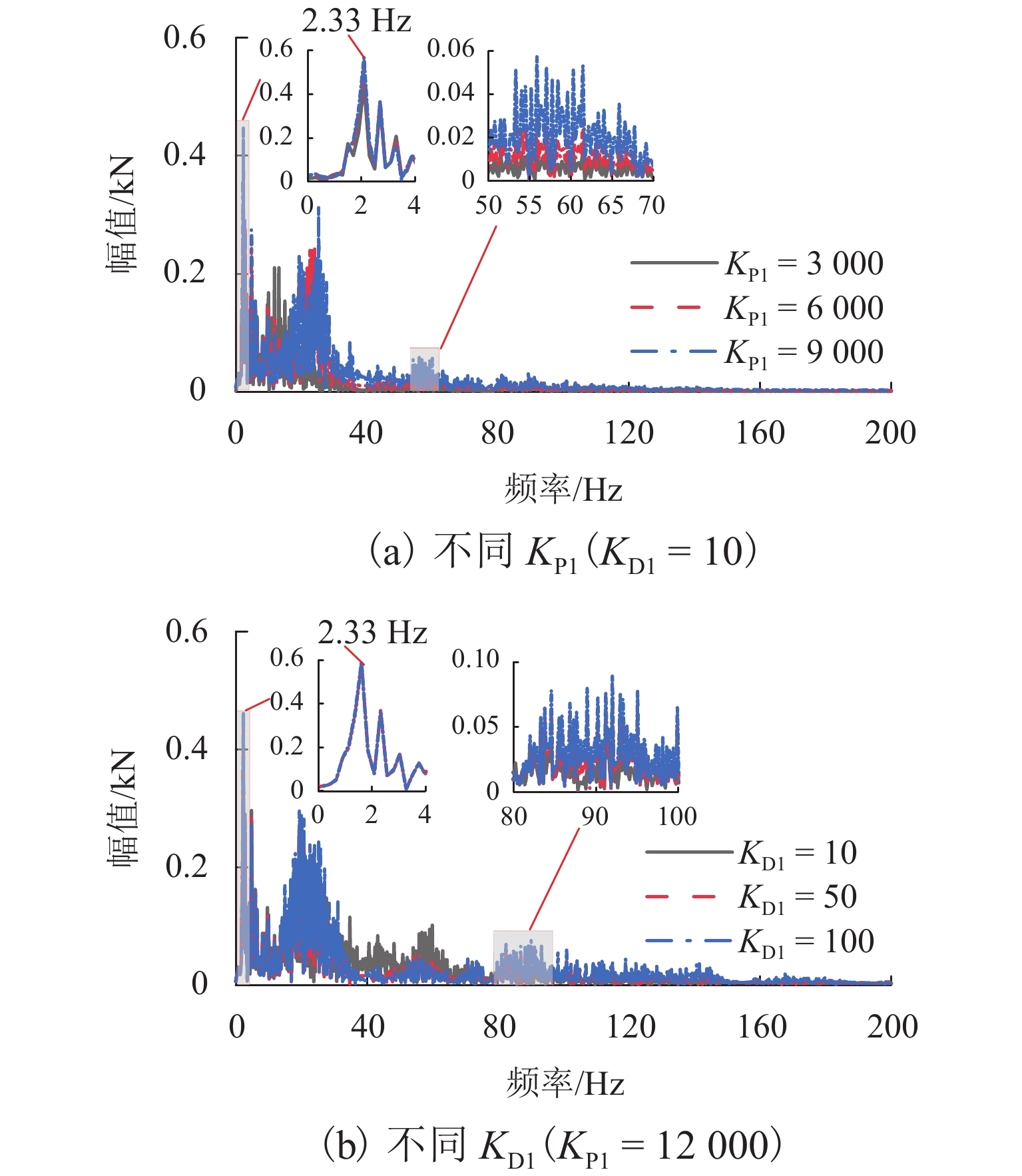
由式(8)可知,增大KP1相当于增大悬浮系统恢复力,而增大KD1相当于增大悬浮系统阻尼力. 为方便分析,图6给出了第12个悬浮电磁铁电磁力最大值随控制参数的变化规律,图7给出了不同控制参数下第12个悬浮电磁铁电磁力的幅值谱. 从图6可以看出:当KP1和KD1较小时,悬浮系统反馈调节未达到饱和,增大KP1或KD1均会引起悬浮电磁力增大,从而导致车体竖向加速度增大; 随着KP1和KD1继续增大,悬浮系统反馈调节逐渐达到饱和,此时车体竖向加速度受KP1和KD1的影响减小;当KP1 > 6000时,由式(9)计算可知,悬浮系统的特征频率大于10.98 Hz,该频率与车体竖向自振频率(2.17 Hz)相差较大. 从图7中可以看出:此时增大KP1或KD1主要是增大电磁力中的高频分量,而高频分量对车体竖向加速度的影响不大;由于KD1相对于KP1数值变化较小,因此增大KD1对车体竖向加速度的增幅较小.
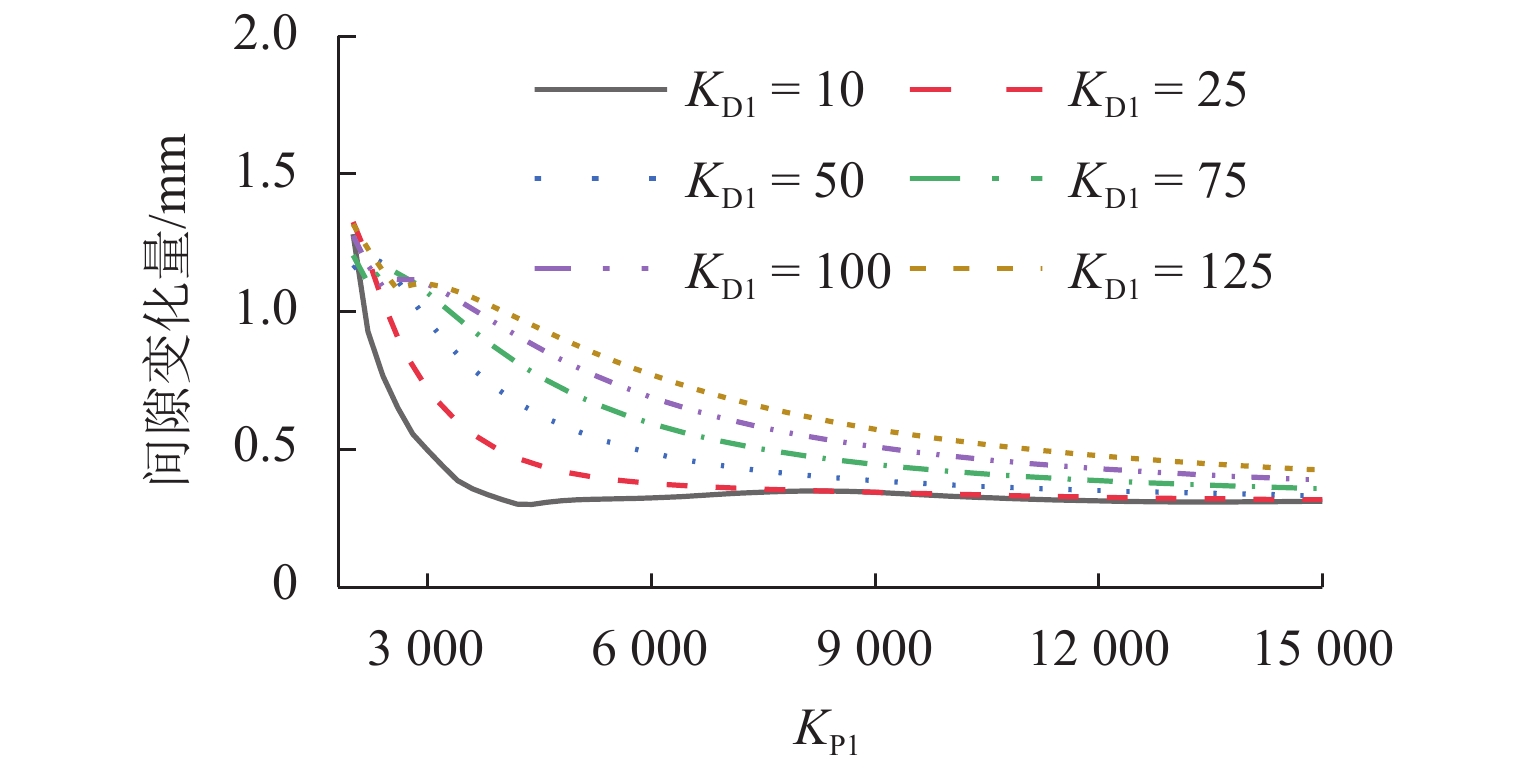
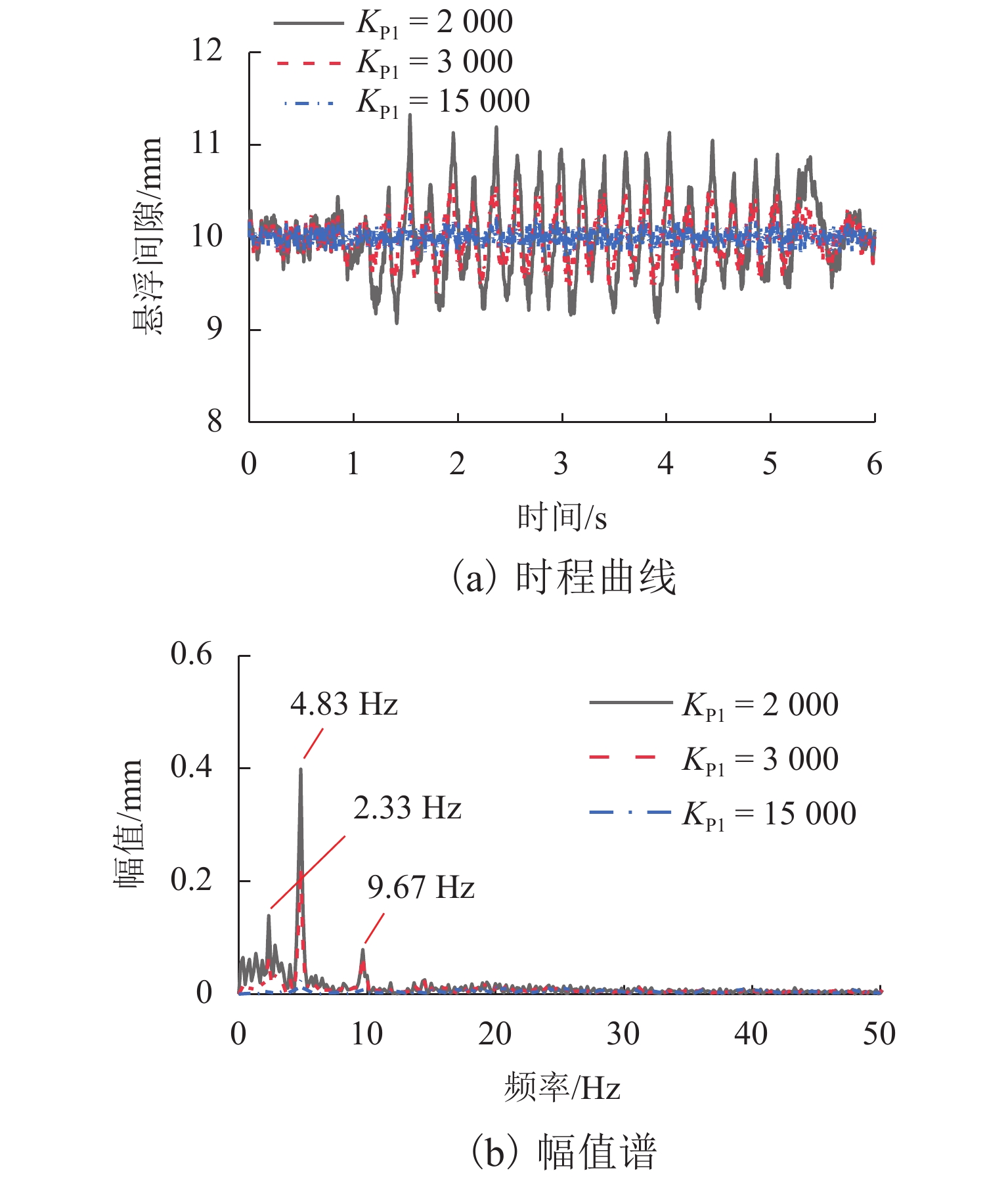
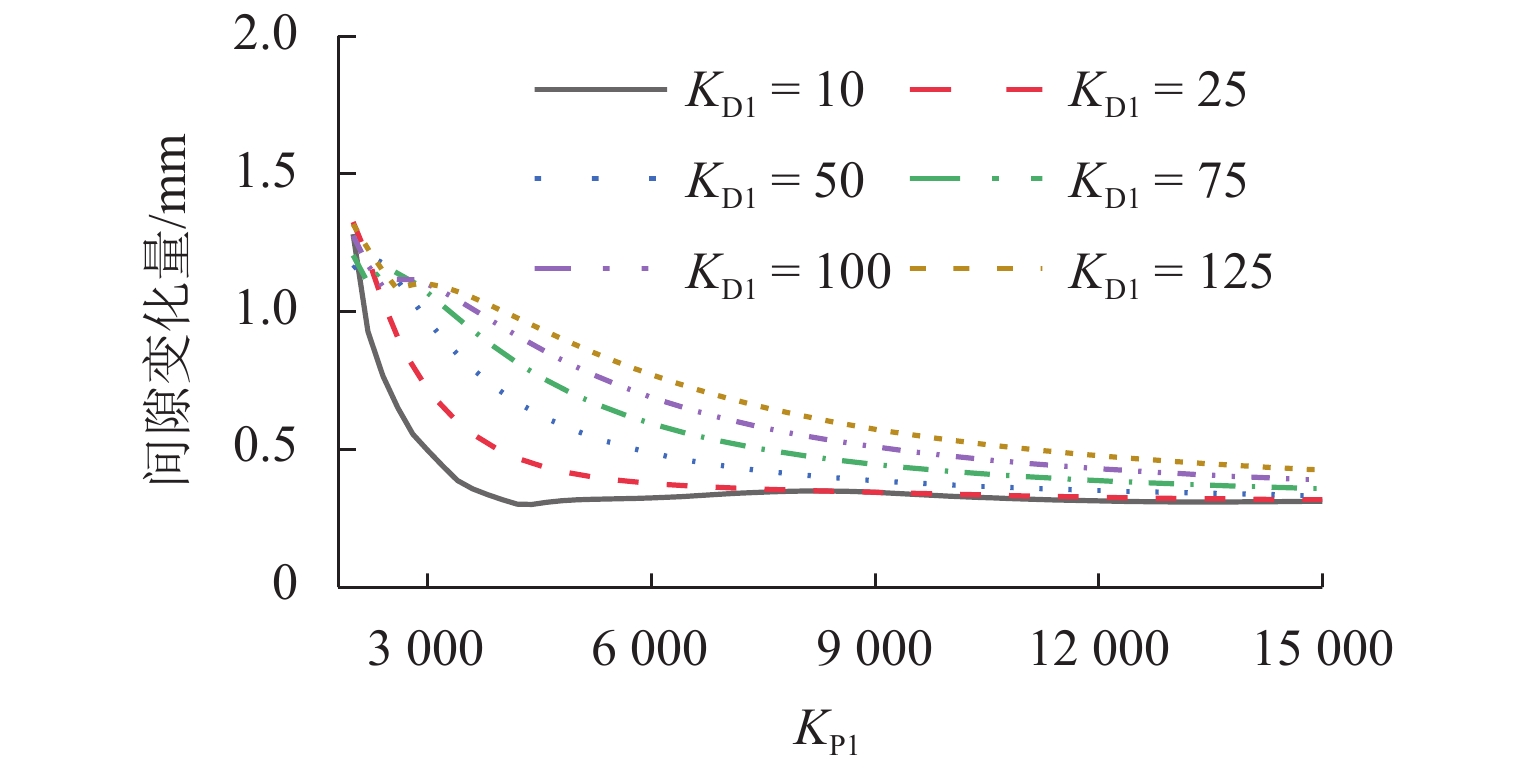
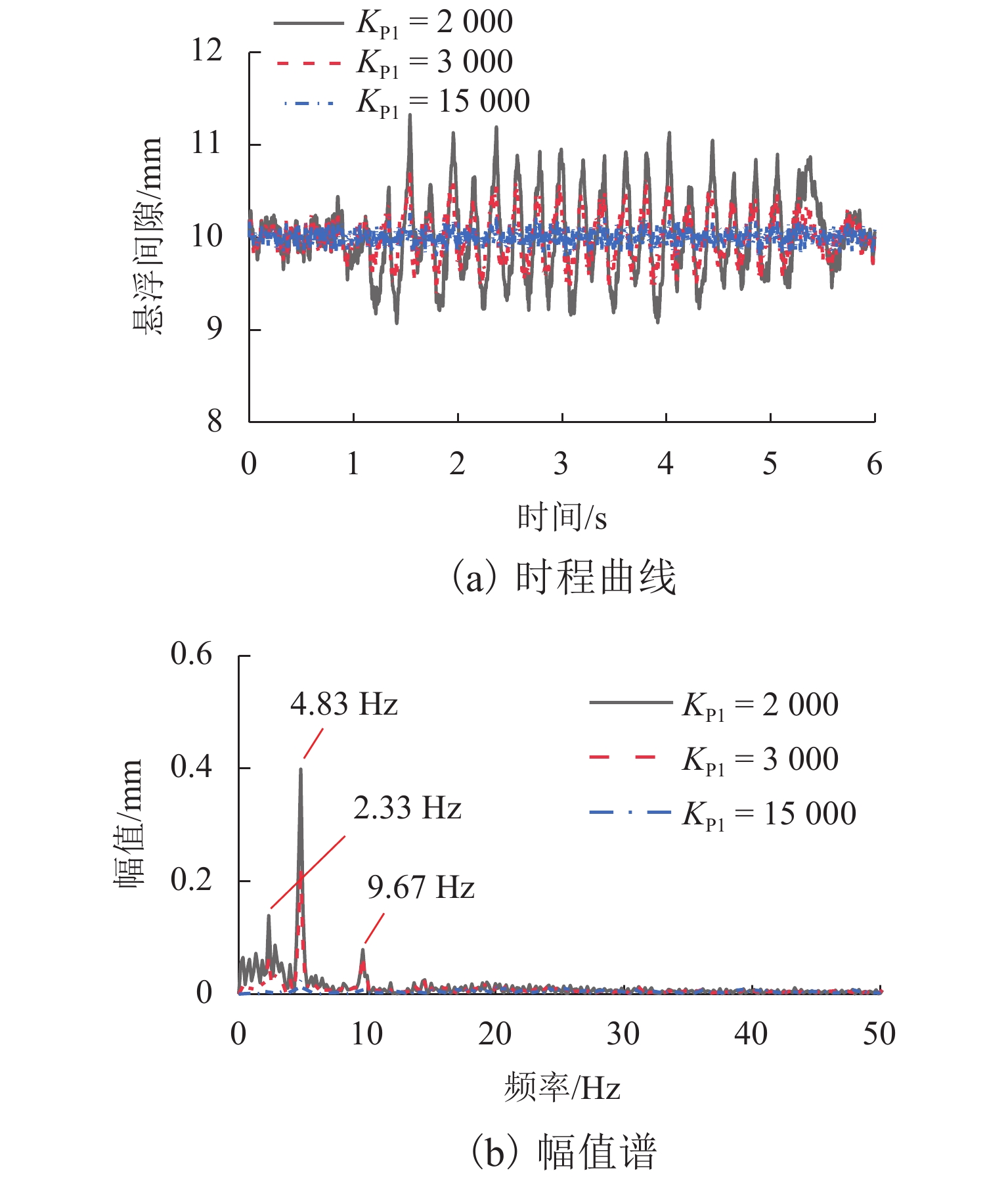
图8为不同控制参数下中间悬浮磁极间隙变化量. 从图8中可以看出:悬浮间隙随着KP1的增大而减小,最后达到一个稳定值,且KD1取值越小,悬浮间隙稳定越快; 同一KP1下,KD1取值越大,悬浮间隙变化量越大. 为进一步分析悬浮间隙随控制参数变化的原因,图9给出了不同控制参数下中间悬浮间隙时程曲线及其幅值谱. 从图9(a)可以看出:当KP1=2000时,列车在桥上段悬浮间隙明显增大,且波动次数刚好等于桥梁的跨数,随着KP1的增大,曲线波动逐渐减小. 从图9(b)可以看出:悬浮间隙幅值谱中存在3个峰值,对应的频率分别为2.33、4.83、9.67 Hz. 由特征频率f计算公式f=v/λ[26]($ \lambda $为特征长度)可以推出,当车速v = 430 km/h时,桥梁跨度24.768 m对应的特征频率为4.82 Hz. 因此,主要频率2.33 Hz对应车体竖向自振频率(2.17 Hz),主要频率4.83 Hz和9.67 Hz分别对应桥梁跨度特征频率(4.82 Hz)及其2倍频(9.64 Hz).
从上述分析中可以得出:当KP1取值较小时,悬浮系统刚度较小,此时悬浮间隙受桥梁动力响应影响较大;随着KP1取值增大,悬浮系统刚度逐渐增大,悬浮间隙受桥梁动力响应的影响逐渐减小,当KP1大于一定值之后,悬浮间隙主要受轨道不平顺的影响,从而悬浮间隙变化量达到一个稳定值;当KP1取值不变,增大KD1时,悬浮系统电磁力增大,导致车-桥系统动力响应的增大,从而悬浮间隙也相应增大.
3.1.2 桥梁动力响应
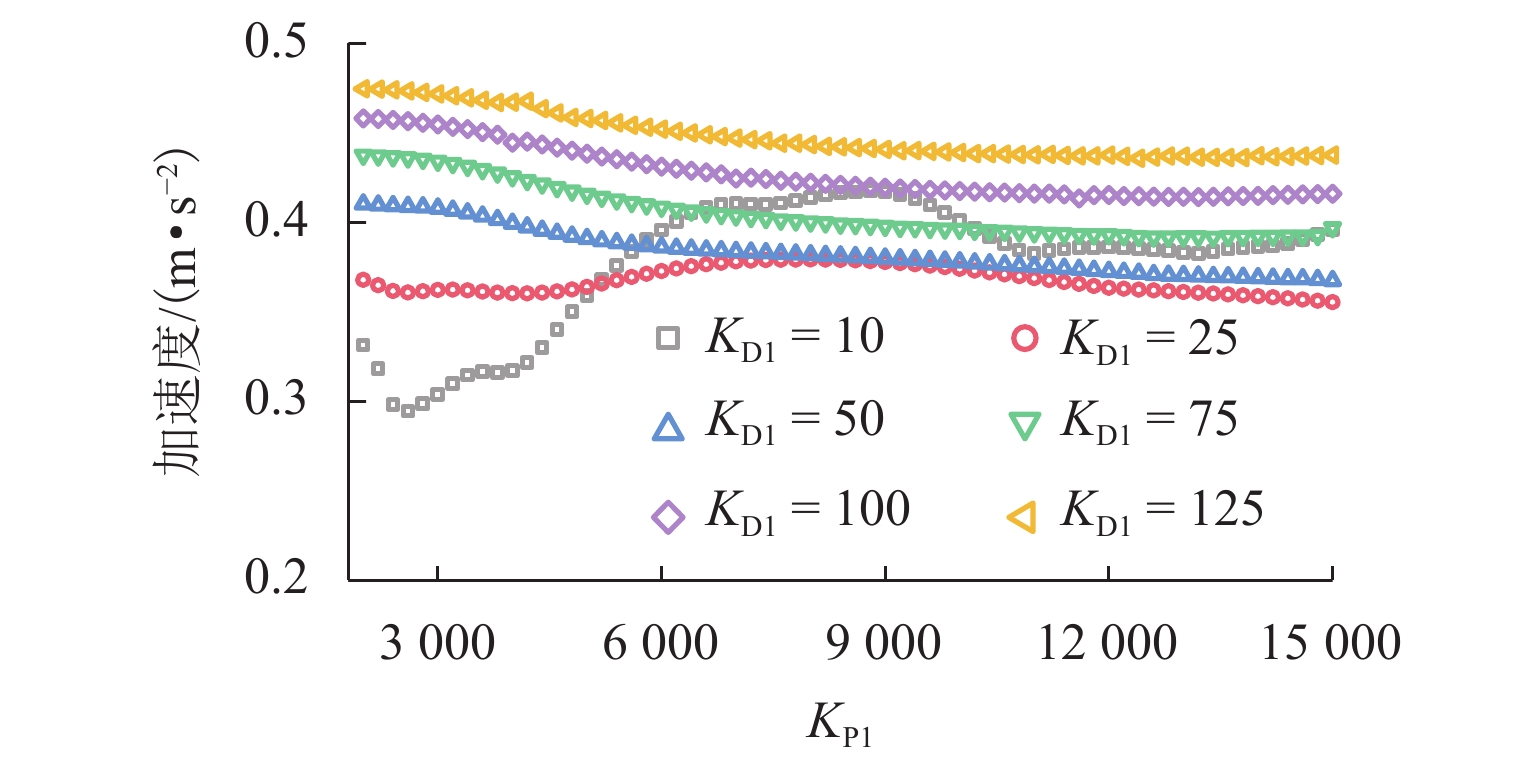
图10中给出了不同控制参数下主梁跨中竖向加速度响应. 从图10中可以看出,当KD1=10时,由式(9)计算得到悬浮系统的阻尼比的范围为0.046~0.335,悬浮系统处于欠阻尼状态,主梁跨中竖向加速度随KP1的增大幅值波动较大;当KD1>25之后,主梁跨中竖向加速度随KP1的增大而减小,同一KP1下,主梁跨中竖向加速度随KD1的增大而增大.
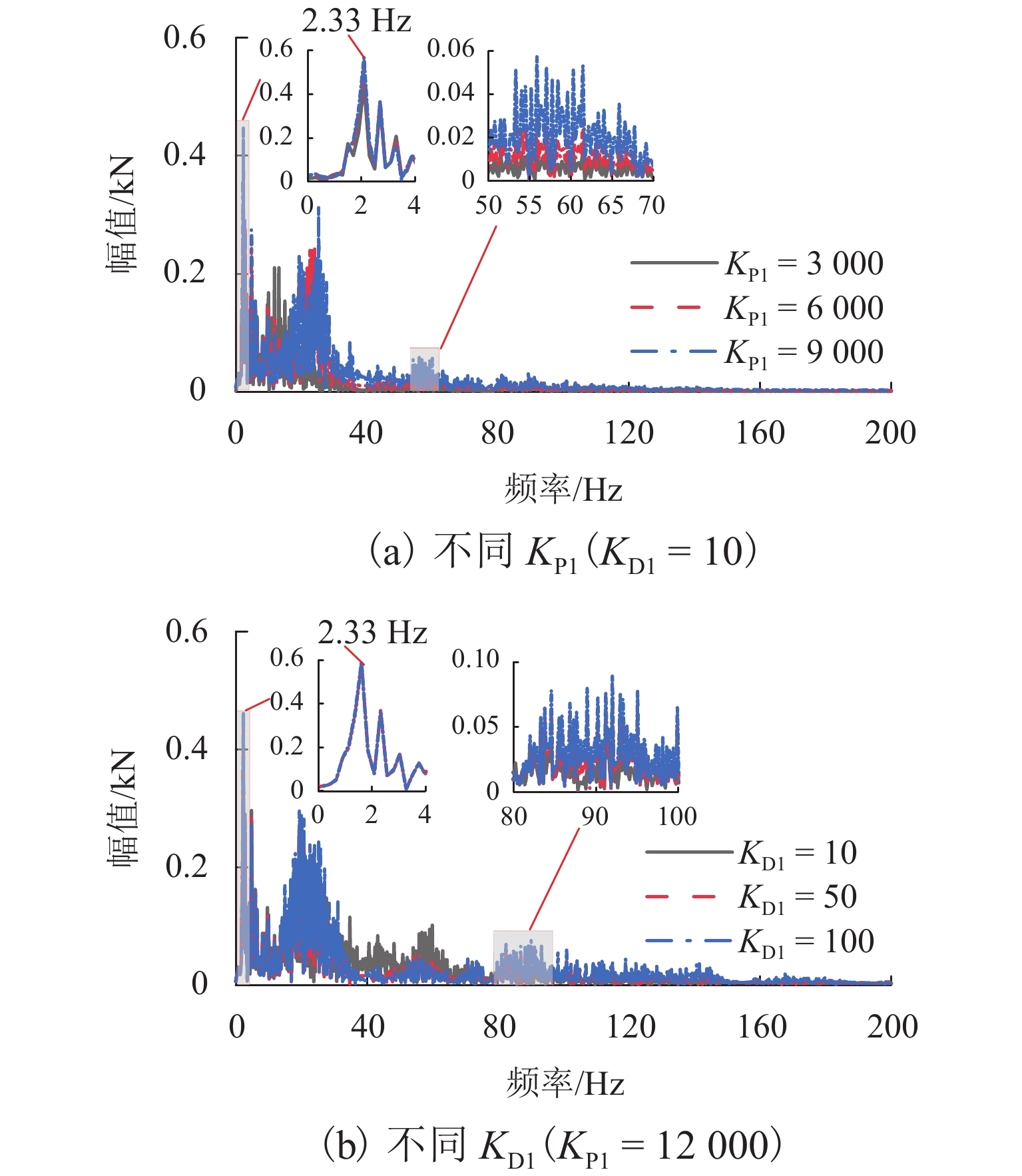
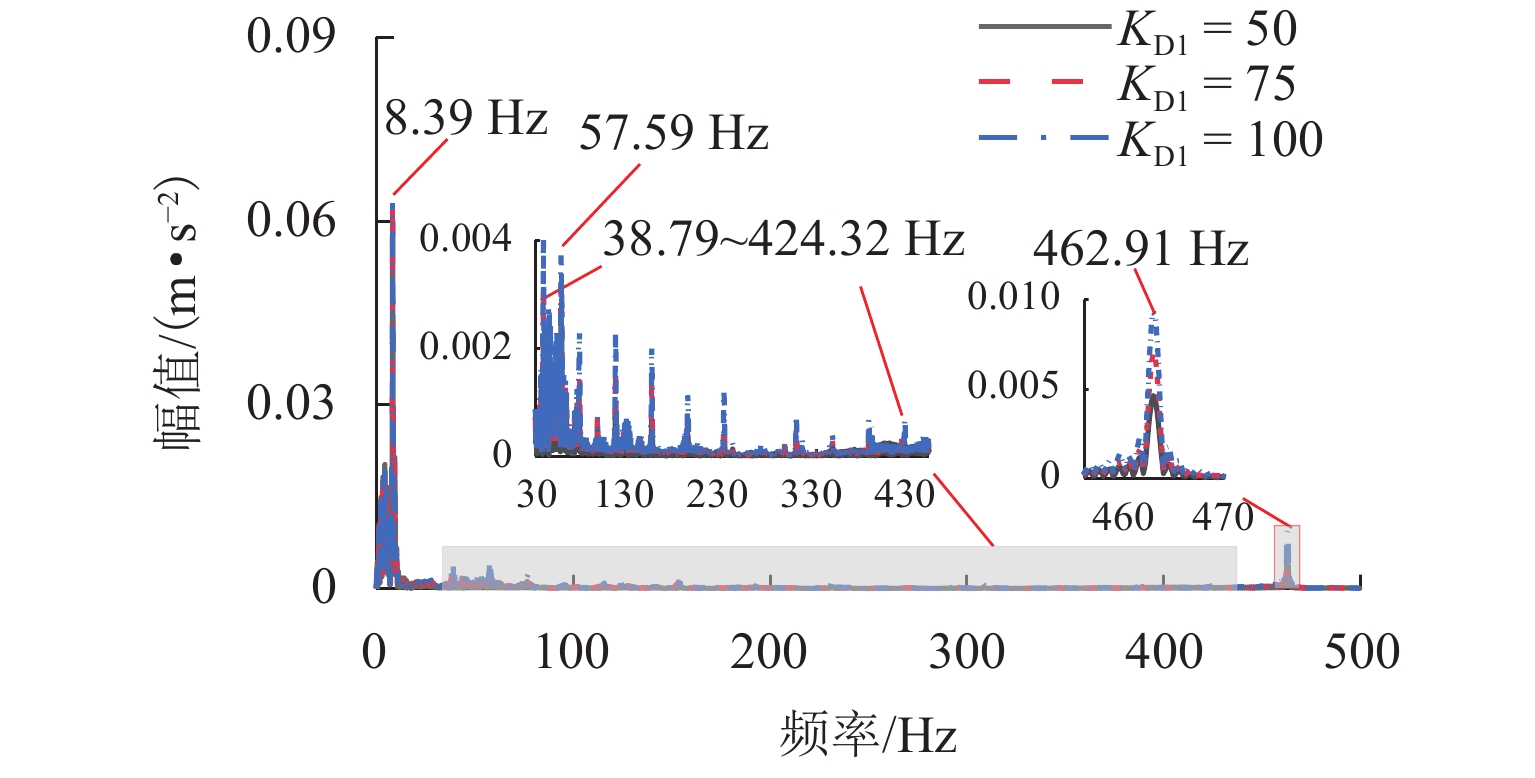
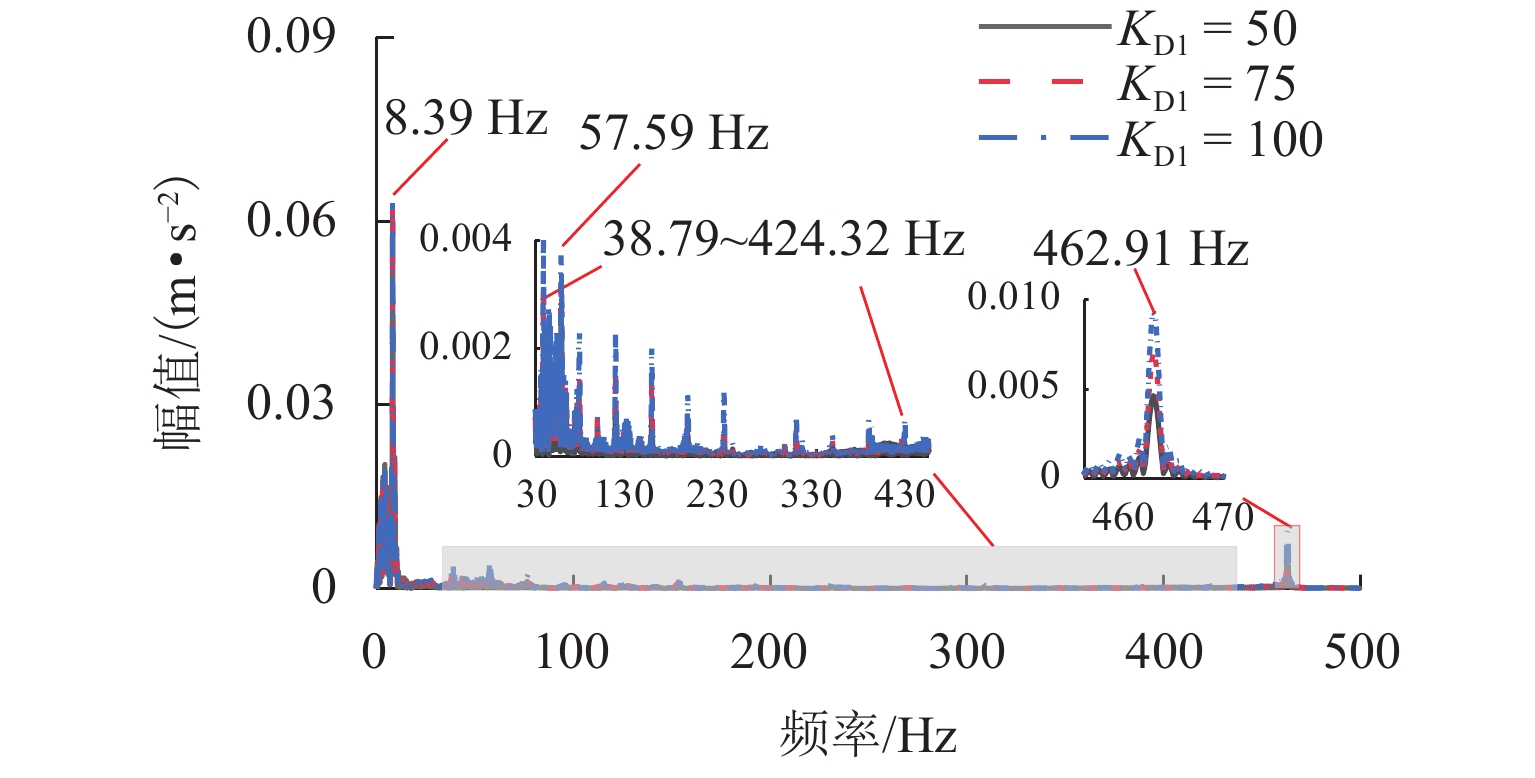
为方便分析,图11给出了不同KD1下主梁跨中竖向加速度的幅值谱. 从图11中可以看出:幅值谱中存在多个峰值,而影响主梁跨中竖向加速度幅值的原因主要有2个,其中一个是主梁自身竖向振动基频,另一个是悬浮电磁铁特征长度(3.096 m)对应特征频率的倍频(1倍~12倍). 1个悬浮电磁铁由12个磁极组成,12倍频刚好是一个悬浮磁极长度(0.258 m,2个激励点之间的距离)对应的特征频率.
主梁跨中竖向加速度幅值谱中的主要频率8.39 Hz和57.59 Hz分别对应主梁的1阶(8.16 Hz)和3阶(57.06 Hz)竖向自振频率. 主要频率38.79~424.32 Hz对应悬浮电磁铁长度特征频率1倍~11倍频(38.58~424.38 Hz),主要频率462.91 Hz对应悬浮电磁铁特征频率的12倍频(462.96 Hz). 从幅值变化看,悬浮电磁铁特征频率倍频下的加速度幅值变化较大,而主梁竖向自振频率下的加速度幅值变化较小,幅值变化最大的是悬浮磁极长度对应的特征频率. 当KP1增大时,悬浮系统刚度增大,悬浮间隙减小,从而导致主梁跨中竖向加速度减小;当KP1不变,KD1增大时,悬浮系统电磁力增大,悬浮间隙增大,从而导致主梁跨中竖向加速度增大.
3.2 横向控制参数
3.2.1 车辆动力响应
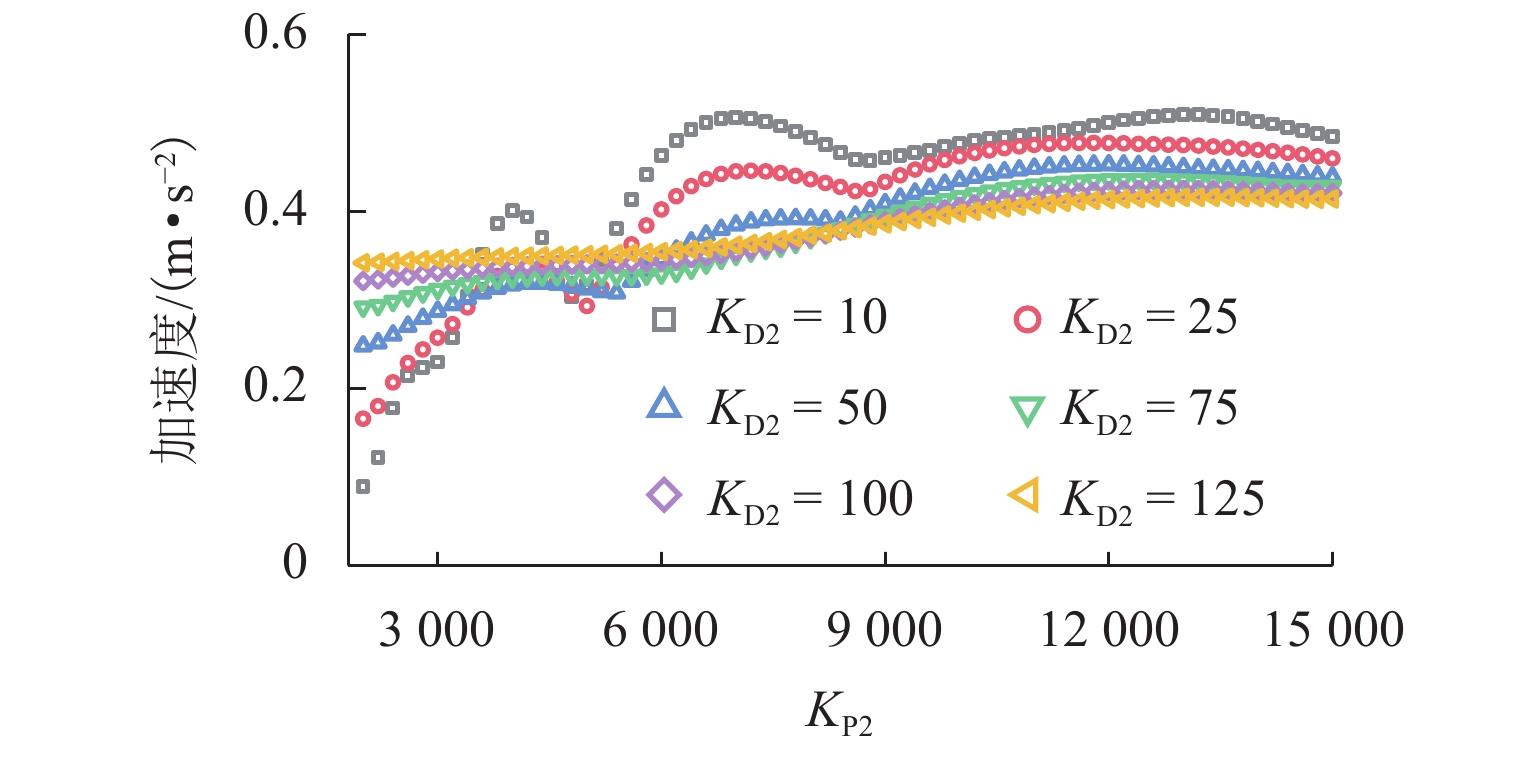
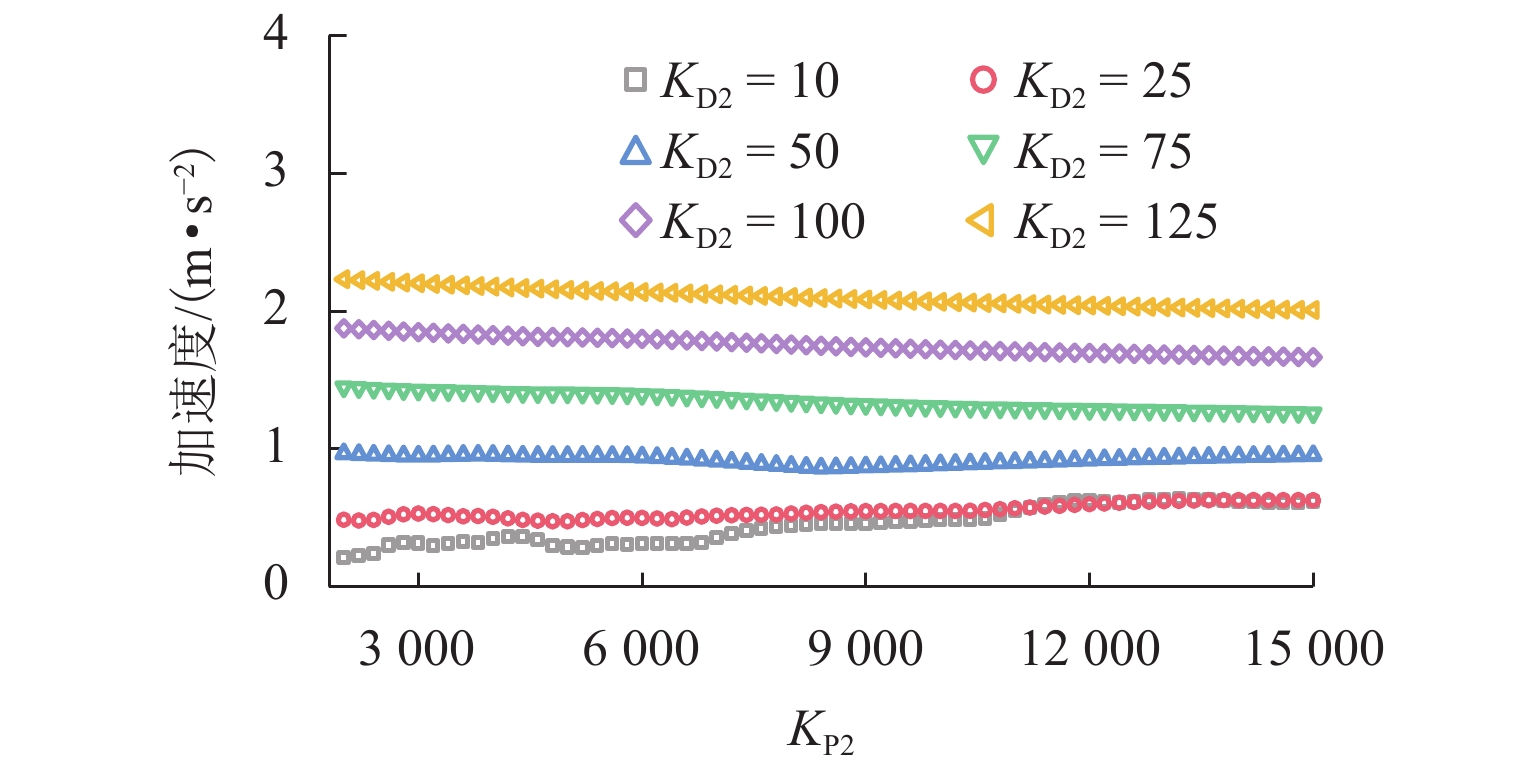
车体横向加速度最大值随控制参数变化如图12所示. 从图12中可以看出:车体横向加速大致随KP2的增大而增大;在KP2逐渐增大的过程中,车体横向加速度从随KD2的增大而增大过渡到了随KD2的增大而减小;当KD2=10时,由式(9)计算得到悬浮系统的阻尼比的范围为0.045~0.423,导向系统图处于欠阻尼状态,因此曲线波动较大.
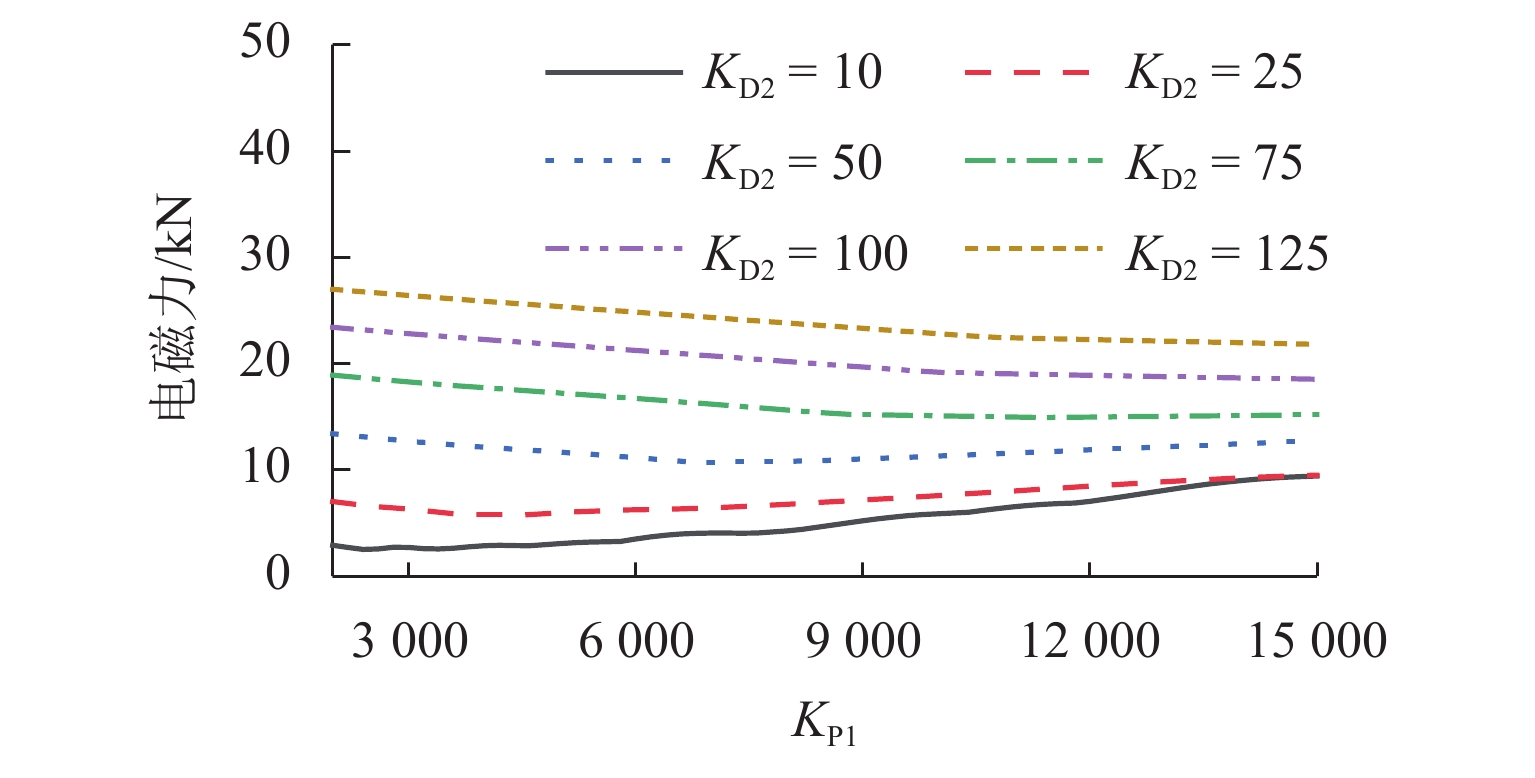
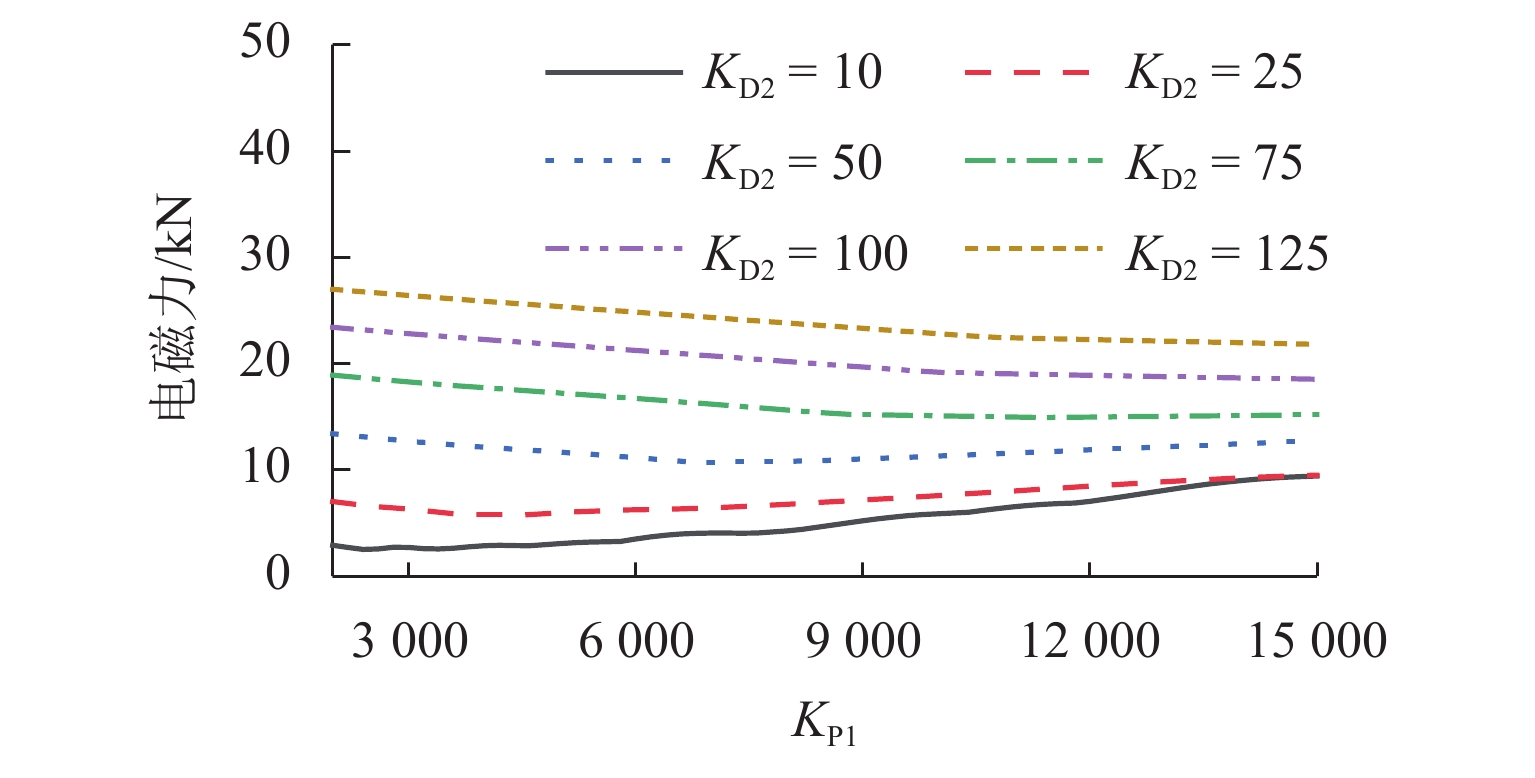
为方便分析,图13给出了不同参数下第12个导向电磁铁的导向电磁力,图14给出了不同控制参数下第12个导向电磁铁电磁力的幅值谱. 从图13中可以看出:导向电磁力随KD2的增大而增大; 在KD2逐渐增大的过程中,导向电磁力从随KP2的增大而增大过渡到了随KP2的增大而减小. 从图14中可以看出:不同控制参数下导向电磁力中高频占比大,低频部分占比小;当KP2取值较小时,导向系统特征频率与车体横向自振频率(5.73 Hz)接近,增大KD2,导向系统的电磁力对车体横向加速度的增幅大于系统阻尼消耗的幅值,因此车体横向加速度会增大;当KP2取值较大时,导向系统特征频率与车体横向自振频率远离,增大KD2,导向系统的电磁力对车体横向加速度的增幅小于系统阻尼消耗的幅值,因此车体横向加速度会减小.
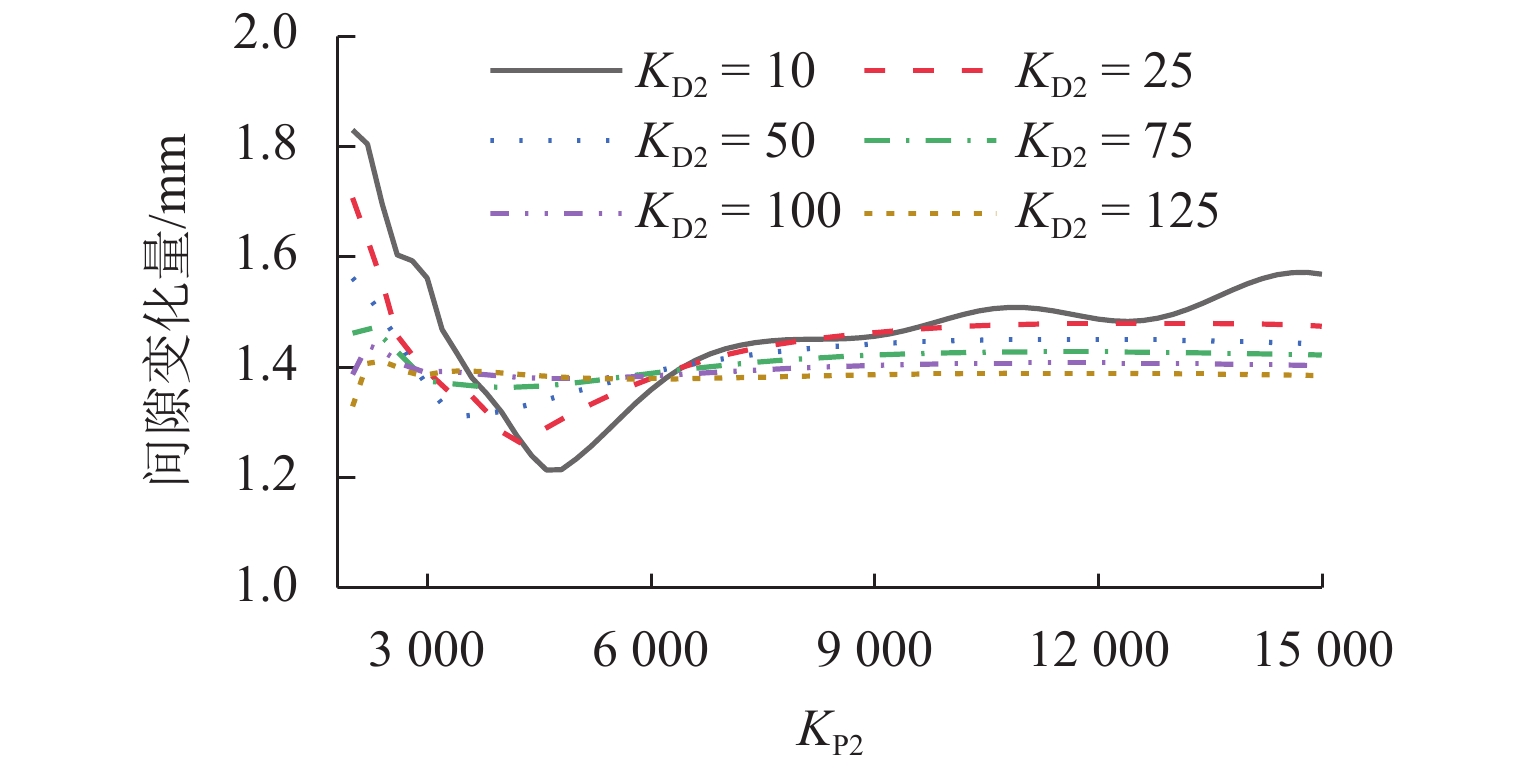
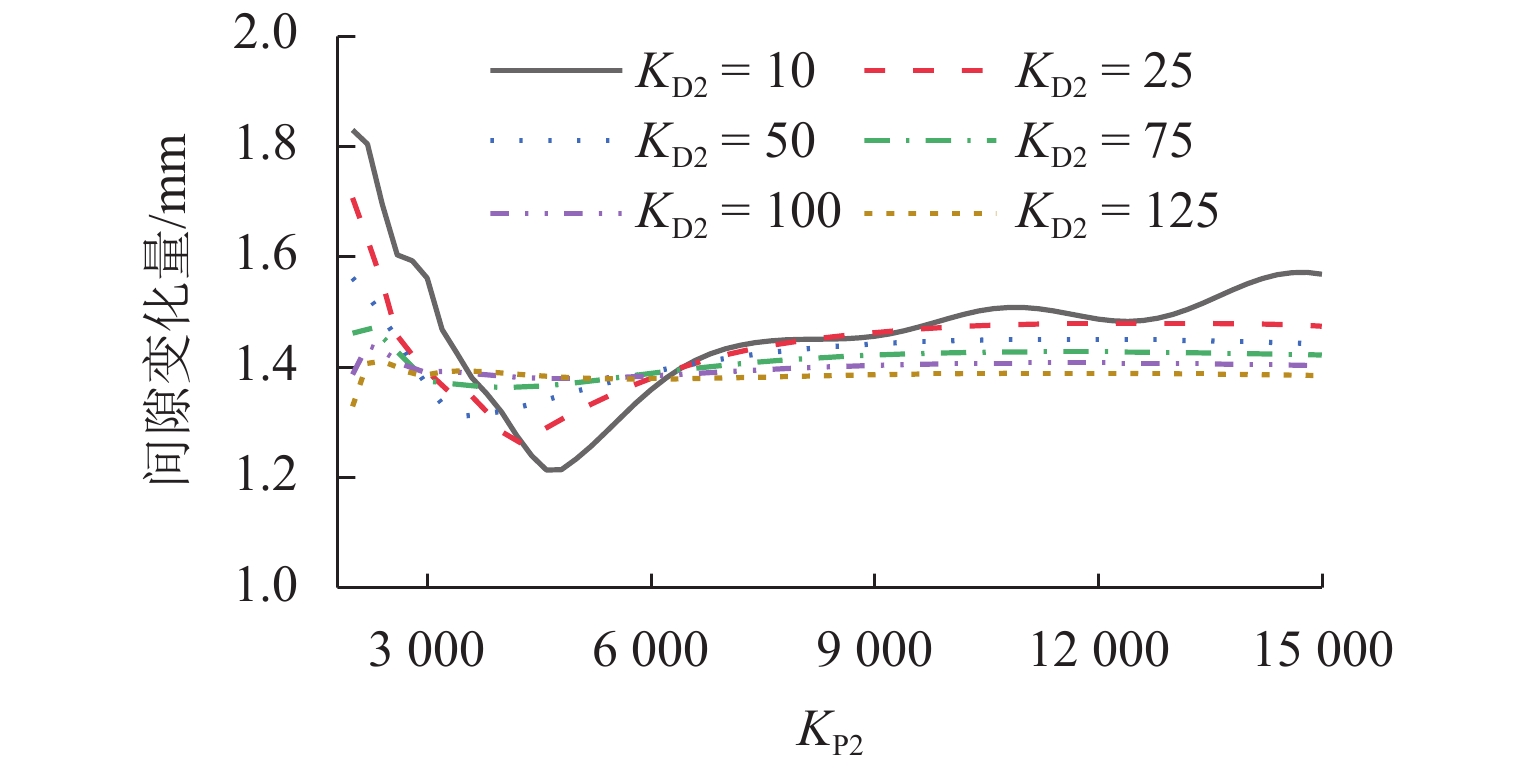
不同控制参数下中间导向磁极间隙变化量如图15所示. 从图15中可以看出:当KD2取值较小时,由于导向系统阻尼较小,导致曲线波动较大,随着KD2取值的增大,导向系统阻尼比增大,KP2对导向间隙变化量的影响逐渐减小,曲线趋于平缓;当KP2取值较大时,由于导向系统反馈调节趋于饱和,增大KD2会导致导向间隙减小.
3.2.2 桥梁动力响应
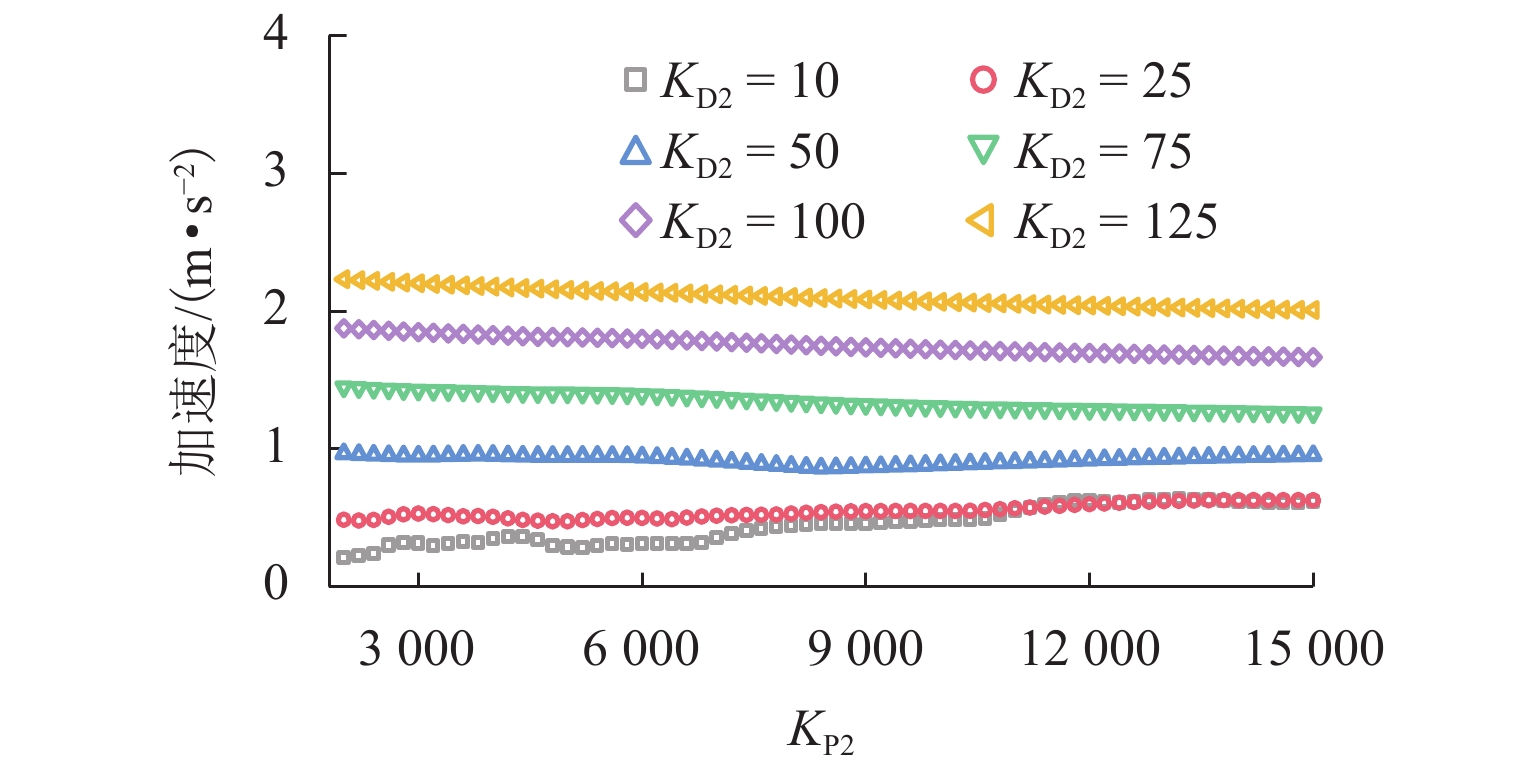
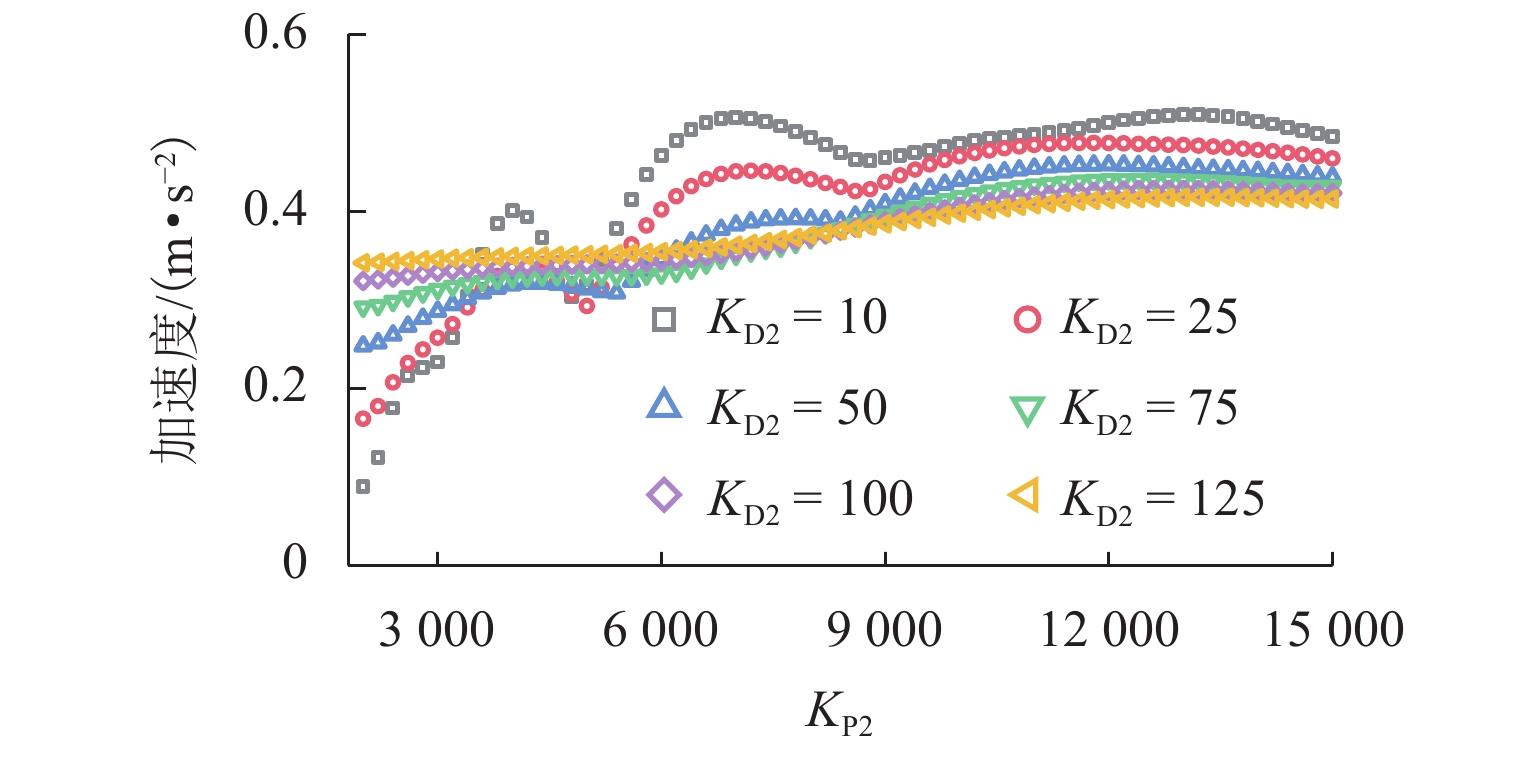
图16给出了不同控制参数下主梁跨中横向加速度最大值. 从图16可以看出:随着KD2的增大,桥梁横向加速度由随KP2增大而增大过渡到随KP2增大而减小;同一KP2下,KD2越大,桥梁横向加速度越大.
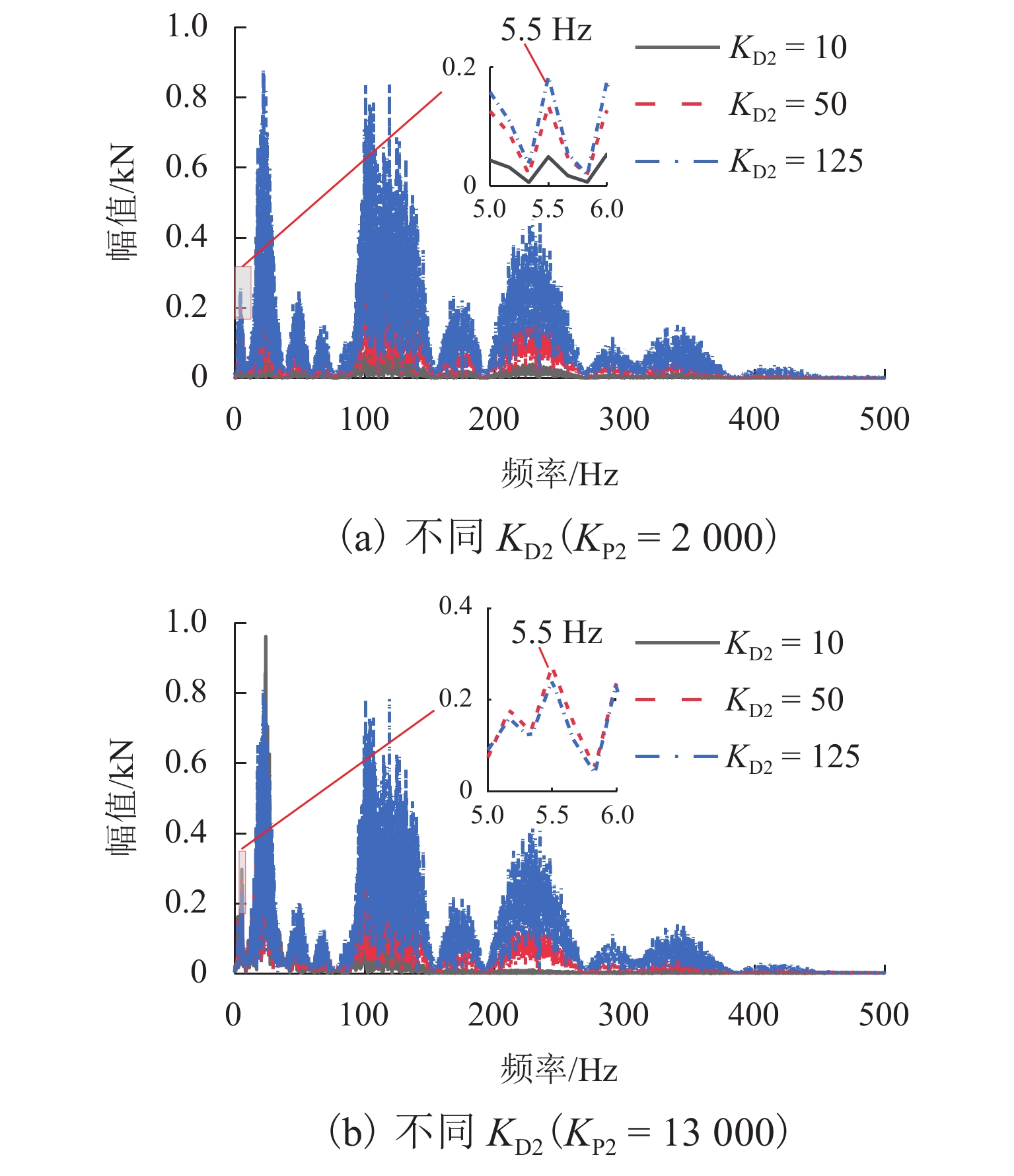
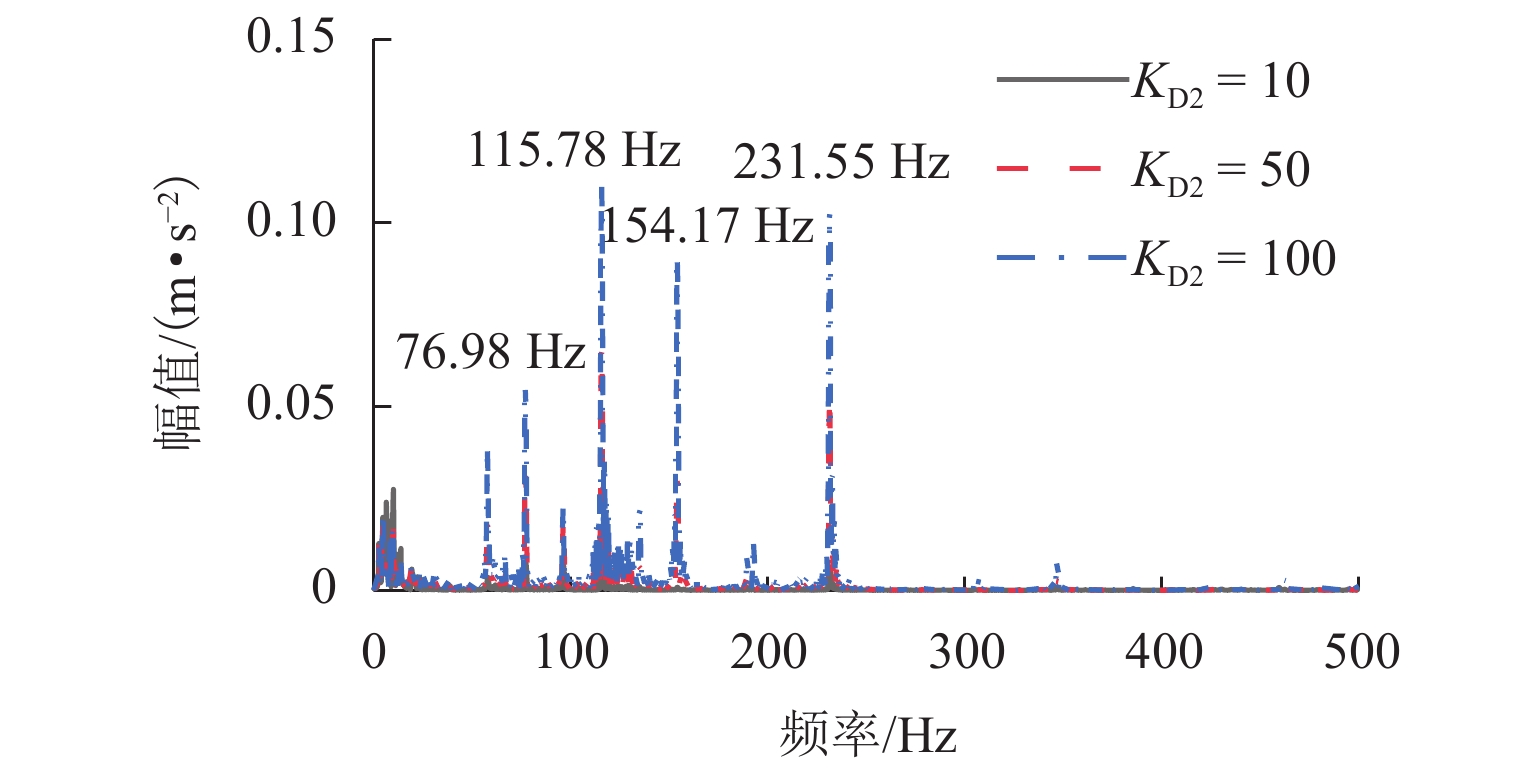
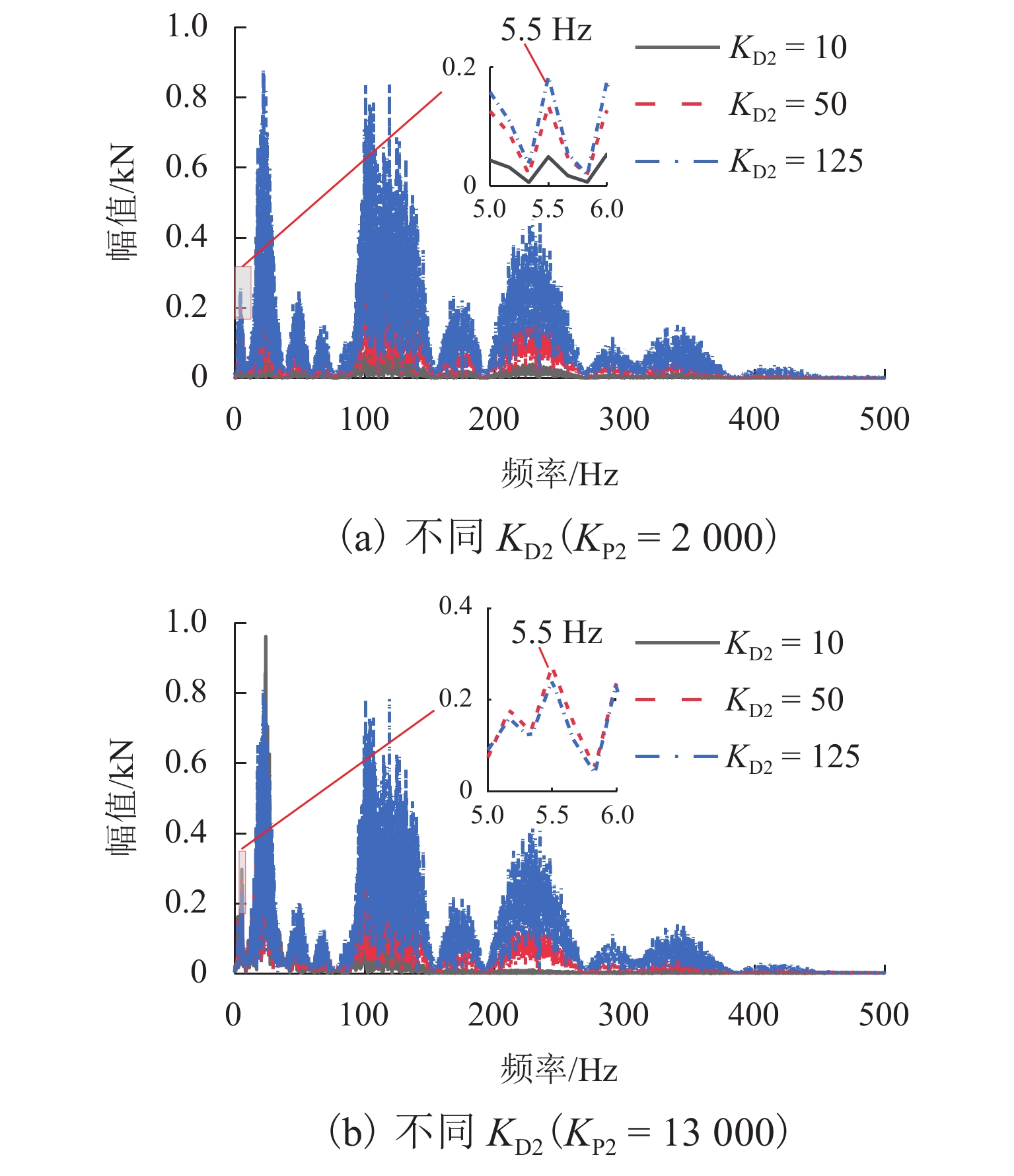
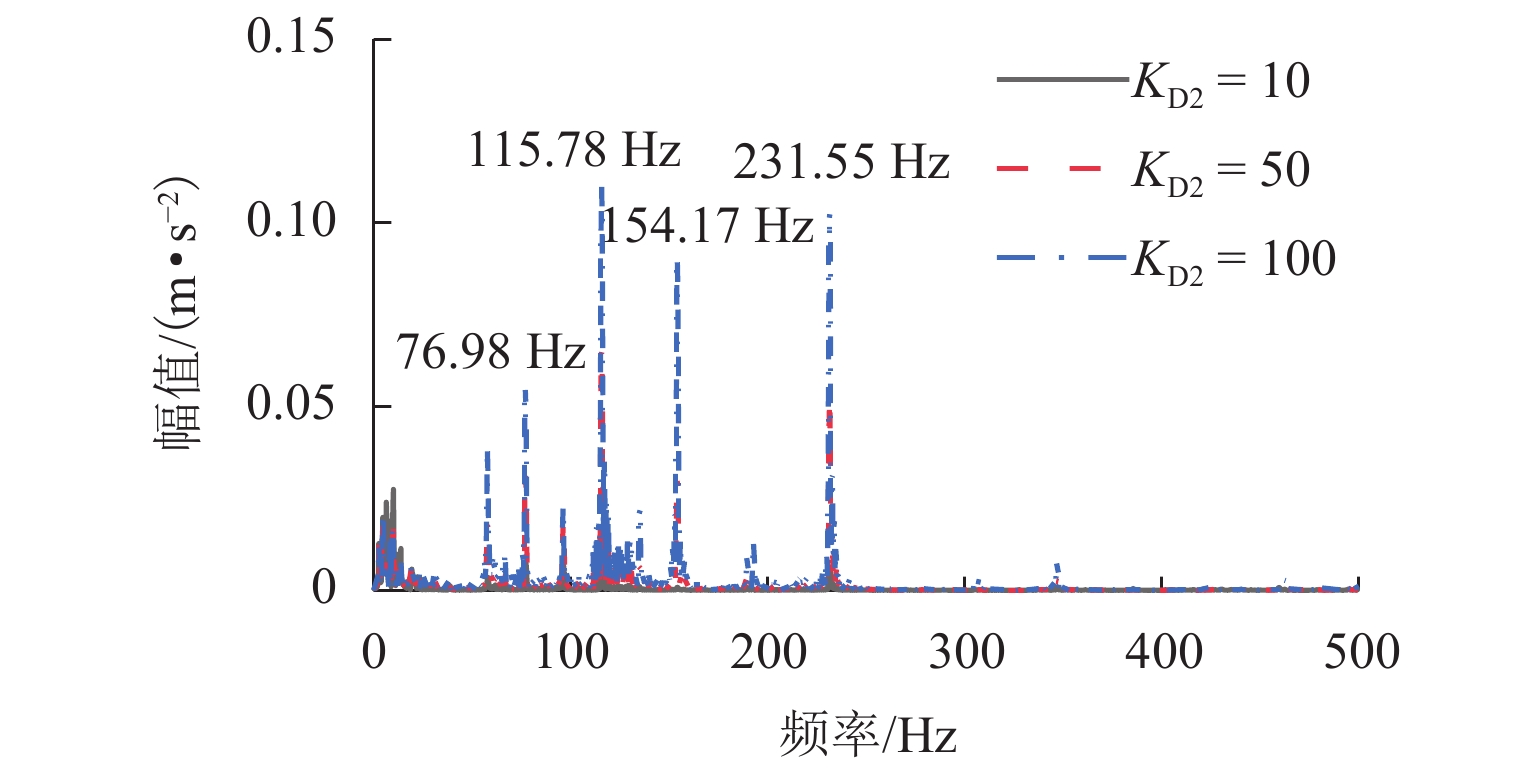
为方便分析,图17给出了不同KD2下主梁跨中横向加速度的幅值谱. 从图17中可以看出:横向加速度幅值谱中主要存在4个峰值,对应的频率分别为76.98、115.78、154.17、231.55 Hz,其中,主要频率115.78 Hz和231.55 Hz分别与导向磁极长度(1.032 m,2个激励点之间的距离)对应的特征频率115.74 Hz及其2倍频231.48 Hz相接近,主要频率76.98 Hz和154.17 Hz分别与导向电磁铁特征长度(3.096 m)对应特征频率38.58 Hz的2倍频77.16 Hz和4倍频154.32 Hz相接近. 从上述分析来看:当KD2较小时,由于导向系统反馈调节没有达到饱和,KP2和KD2的增大都会导致电磁力增大,从而导致桥梁加速度增大;当KD2较大时,导向系统调节达到饱和,此时增大KP2,导向系统刚度增大,导向电磁铁响应减小,从而导致桥梁加速度减小.
4. 结 论
本文以上海高速磁浮为背景,开展了430 km/h车速下不同控制参数对磁浮车-桥耦合系统动力响应的影响分析,主要结论如下:
1) KP1<6000时,增大KP1或KD1悬浮电磁力均会增大,从而导致车体竖向加速度增大. KP1>6000时,悬浮系统反馈调节逐渐达到饱和,车体竖向加速度随KP1的增大先增大后平稳. 同理,增大KP2导向电磁力增大,车体横向加速也相应增大.
2) 随着KP1的增大,悬浮系统刚度亦增大,悬浮间隙受桥梁跨度特征频率的影响逐渐减小. 悬浮间隙随KP1的增大而减小,最后趋于稳定. 增大KD1时,悬浮电磁力增大,从而导致悬浮间隙增大. 由于导向系统本身刚度较大,因此受KP2的影响较小,且KD2越大,影响越小.
3) 桥梁竖向加速度随KP1的增大而减小,随KD1的增大而增大. 在KD2的过程中,桥梁横向加速度由随KP2增大而增大过渡到随KP2增大而减小. 同一KP2下,KD2越大,桥梁横向加速度越大. 桥梁竖向加速度主要受电磁力中悬浮电磁铁长度特征频率1 倍~12倍频的影响,桥梁横向加速度主要受导向磁极长度特征频率及其2倍频和导向电磁铁长度特征频率2倍频及4倍频的影响.
4) 为使车桥系统动力响应尽可能小,综合建议竖向控制参数KP1和KD1的取值范围分别为3000~4000和10~25,横向控制参数KP2和KD2的取值范围分别为4000~5000和10~25.
致谢:长沙理工大学桥梁工程安全控制教育部重点实验室开放基金(22KB02).
-
表 1 车辆模型参数
Table 1. Parameters of train model
参数 数值 车体质量/(× 104 kg) 3.90 车体侧滚质量惯性矩/(× 104 kg•m2) 6.46 车体摇头质量惯性矩/(× 104 kg•m2) 1.75 车体点头质量惯性矩/(× 104 kg•m2) 1.76 一系/二系弹簧竖向刚度/(×106 N•m−1) 20/2 一系/二系弹簧竖向阻尼/(×103 N•s•m−1) 5/5 一系/二系弹簧横向刚度/(×106 N•m−1) 28/2 一系/二系弹簧横向阻尼/(×102 N•s•m−1) 5/20 空气弹簧刚度竖向/(× 106 N•m−1) 1.90 表 2 桥梁模型参数
Table 2. Parameters of bridge model
参数 数值 主梁/钢轨/桥墩密度/(kg•m−3) 2551/7850/2500 主梁/钢轨/桥墩弹性模量/GPa 44.5/206.0/30.0 主梁/钢轨/桥墩泊松比 0.2/0.3/0.2 -
[1] SU X Y, XU Y L, YANG X F. Neural network adaptive sliding mode control without overestimation for a maglev system[J]. Mechanical Systems and Signal Processing, 2022, 168: 108661.1-108661.12. [2] 马卫华,胡俊雄,李铁,等. EMS型中低速磁浮列车悬浮架技术研究综述[J]. 西南交通大学学报,2023,58(4): 720-733.MA Weihua, HU Junxiong, LI Tie, et al. Technologies research review of electro-magnetic suspension medium-low-speed maglev train levitation frame[J]. Journal of Southwest Jiaotong University, 2023, 58(4): 720-733. [3] 熊嘉阳,邓自刚. 高速磁悬浮轨道交通研究进展[J]. 交通运输工程学报,2021,21(1): 177-198.XIONG Jiayang, DENG Zigang. Research progress of high-speed maglev rail transit[J]. Journal of Traffic and Transportation Engineering, 2021, 21(1): 177-198. [4] WANG L D, BU X M, HU P, et al. Dynamic reliability analysis of running safety and stability of a high-speed maglev train on a guideway bridge[J]. International Journal of Structural Stability and Dynamics, 2023: 1-41. [5] 翟明达,朱朋博,李晓龙,等. 常导电磁型高速磁浮列车主动导向能力的评估与验证[J]. 西南交通大学学报,2022,57(3): 514-521.ZHAI Mingda, ZHU Pengbo, LI Xiaolong, et al. Evaluation and verification for active guidance ability of EMS maglev train[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 514-521. [6] 陆海英,韩霄翰,李忠继,等. 中低速磁浮系统起浮阶段的振动特性分析[J]. 中国机械工程,2019,30(3): 318-324.LU Haiying, HAN Xiaohan, LI Zhongji, et al. Analysis of vibration characteristics of low-medium speed maglev levitation systems in lifting stages[J]. China Mechanical Engineering, 2019, 30(3): 318-324. [7] 翟婉明,赵春发. 现代轨道交通工程科技前沿与挑战[J]. 西南交通大学学报,2016,51(2): 209-226.ZHAI Wanming, ZHAO Chunfa. Frontiers and challenges of sciences and technologies in modern railway engineering[J]. Journal of Southwest Jiaotong University, 2016, 51(2): 209-226. [8] 韩霄翰,李忠继,池茂儒. 轨道梁结构对中低速磁浮车轨耦合振动的影响[J]. 铁道机车车辆,2019,39(5): 36-42.HAN Xiaohan, LI Zhongji, CHI Maoru. Influence of track beam structure on the mid-low maglev vehicle-rail coupling vibration[J]. Railway Locomotive & Car, 2019, 39(5): 36-42. [9] FENG Y, ZHAO C F, WU D H, et al. Effect of levitation gap feedback time delay on the EMS maglev vehicle system dynamic response[J]. Nonlinear Dynamics, 2023, 111(8): 7137-7156. doi: 10.1007/s11071-022-08225-5 [10] 赵春发,翟婉明. 常导电磁悬浮动态特性研究[J]. 西南交通大学学报,2004,39(4): 464-468.ZHAO Chunfa, ZHAI Wanming. Dynamic characteristics of electromagnetic levitation systems[J]. Journal of Southwest Jiaotong University, 2004, 39(4): 464-468. [11] 蔡文涛,王春江,滕念管,等. 高速磁浮双向主动控制系统模型参数分析[J]. 力学季刊,2020,41(3): 477-485.CAI Wentao, WANG Chunjiang, TENG Nianguan, et al. Study on the parameters of bi-directional active control system for high speed maglev[J]. Chinese Quarterly of Mechanics, 2020, 41(3): 477-485. [12] 梁鑫,罗世辉,马卫华. 单磁铁悬浮控制系统反馈参数动力学特性分析[J]. 噪声与振动控制,2012,32(5): 62-66,135.LIANG Xin, LUO Shihui, MA Weihua. Dynamic characteristics of feedback coefficient of single magnet suspension control system[J]. Noise and Vibration Control, 2012, 32(5): 62-66,135. [13] 汤港归,汪斌,夏翠鹏,等. 磁浮车辆起浮参数控制及其动力性能研究[J]. 铁道科学与工程学报,2023,20(3): 790-801.TANG Ganggui, WANG Bin, XIA Cuipeng, et al. Control of levitation parameters and dynamic performance of maglev vehicle[J]. Journal of Railway Science and Engineering, 2023, 20(3): 790-801. [14] SUN Y G, HE Z Y, XU J Q, et al. Dynamic analysis and vibration control for a maglev vehicle-guideway coupling system with experimental verification[J]. Mechanical Systems and Signal Processing, 2023, 188: 109954.1-109954.18. [15] 杨志南,冯洋,刘东生,等. 悬浮控制参数对磁浮车辆与道岔梁耦合振动的影响分析[J]. 机械,2022,49(2): 38-46.YANG Zhinan, FENG Yang, LIU Dongsheng, et al. Influence of levitation control parameters on coupled vibration between maglev vehicles and switch girder[J]. Machinery, 2022, 49(2): 38-46. [16] XIANG H Y, TIAN X F, LI Y L, et al. Dynamic interaction analysis of high-speed maglev train and guideway with a control loop failure[J]. International Journal of Structural Stability and Dynamics, 2022, 22(10): 2241012.1-224101228. [17] GUO W, CHEN X Y, YE Y T, et al. Coupling vibration analysis of high-speed maglev train-viaduct systems with control loop failure[J]. Journal of Central South University, 2022, 29(8): 2771-2790. doi: 10.1007/s11771-022-5119-1 [18] 谢云德,常文森. 电磁型(EMS)磁悬浮列车系统铅垂方向的建模与仿真[J]. 铁道学报,1996,18(4): 47-54.XIE Yunde, CHANG Wensen. Modeling and simulation of electromagnetic (EMS) maglev train system in vertical direction[J]. Journal of the China Railway Society, 1996, 18(4): 47-54. [19] ZHANG L, HUANG J Y. Dynamic interaction analysis of the high-speed maglev vehicle/guideway system based on a field measurement and model updating method[J]. Engineering Structures, 2019, 180: 1-17. doi: 10.1016/j.engstruct.2018.11.031 [20] 张宝安,虞大联,李海涛,等. 高速磁浮悬浮架柔性特征对曲线通过性能的影响[J]. 西南交通大学学报,2022,57(3): 475-482.ZHANG Baoan, YU Dalian, LI Haitao, et al. Influence of flexibility characteristics of levitation chassis on curve negotiation performance of high-speed maglev vehicle[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 475-482. [21] ZHAO C F, ZHAI W M. Maglev vehicle/guideway vertical random response and ride quality[J]. Vehicle System Dynamics, 2002, 38(3): 185-210. doi: 10.1076/vesd.38.3.185.8289 [22] SHI J, FANG W S, WANG Y J, et al. Measurements and analysis of track irregularities on high speed maglev lines[J]. Journal of Zhejiang University-Science A, 2014, 15(6): 385-394. doi: 10.1631/jzus.A1300163 [23] WANG Z L, XU Y L, LI G Q, et al. Modelling and validation of coupled high-speed maglev train-and-viaduct systems considering support flexibility[J]. Vehicle System Dynamics, 2019, 57(2): 161-191. doi: 10.1080/00423114.2018.1450517 [24] SHI J, WANG Y J. Dynamic response analysis of single-span guideway caused by high speed maglev train[J]. Latin American Journal of Solids and Structures, 2011, 8(3): 213-228. doi: 10.1590/S1679-78252011000300001 [25] XU Y L, WANG Z L, LI G Q, et al. High-speed running maglev trains interacting with elastic transitional viaducts[J]. Engineering Structures, 2019, 183: 562-578. doi: 10.1016/j.engstruct.2019.01.012 [26] 徐金辉,王平,汪力,等. 基于频域分析方法的轨道高低不平顺敏感波长的研究[J]. 中南大学学报(自然科学版),2016,47(2): 683-689.XU Jinhui, WANG Ping, WANG Li, et al. Sensitive wavelengths of vertical track irregularities by frequency-domain method[J]. Journal of Central South University (Science and Technology), 2016, 47(2): 683-689. -






 下载:
下载:
















 下载:
下载: