Modeling and Robust Control of Magnetic Bearing-Rotor System Considering Interface Contact
-
摘要:
磁悬浮轴承-转子系统中，螺栓连接装配形成的界面接触会在转子悬浮状态下激发弯曲模态振动，同时振动频率随转速明显变化. 为实现全转速范围内对弯曲模态振动的主动控制，提出一种考虑频率不确定性的H∞鲁棒控制器设计方法. 首先，通过建立考虑界面接触的动力学模型进行仿真预测，获得振动频率的变化范围；其次，通过频响拟合的方式对转子传递函数进行重构，并将仿真得到的振动频率变化范围以加性不确定性方式引入到重构传递函数中，得到考虑模态频率不确定性的被控对象模型；最后，基于该模型设计兼顾参数摄动和外力扰动的鲁棒性、闭环系统稳定性和防止控制电压饱和等多功能的H∞ 鲁棒控制器. 数值仿真结果表明：该控制器在模态频率处具有宽频带阻的频响特性，能够抑制磁悬浮轴承转子系统的弯曲模态振动；采用该方法设计的H∞ 鲁棒控制器后，转子弯曲模态振动幅值最少减小90%.
Abstract:In magnetic bearing-rotor systems, the bending mode vibration may be excited by the interface contact formed by bolt joints during rotor levitation, and the vibration frequency varies with rotation speed. To actively control bending mode vibration at any speed, the design method of a robust H∞ controller considering frequency uncertainty was proposed. Firstly, the dynamic model considering interface contact was established for numerical simulation, and the vibration frequency variation was obtained. Then, the rotor transfer function was reconstructed by frequency response fitting, and the variation range of vibration frequency obtained by simulation was introduced into the reconstructed transfer function by means of additive uncertainty. As a result, a controlled object model considering mode frequency uncertainty was obtained. Finally, based on the model, the robust H∞ controller was designed by taking the robustness to parameter perturbations and external disturbance, closed-loop system stability, control voltage saturation, and other functions into account. The numerical simulation results show that the controller has the frequency response characteristic of wide band resistance at the mode frequency, which is able to suppress the bending mode vibration of the magnetic bearing-rotor system. After the robust H∞ controller designed by this method is used, the bending mode vibration amplitude of the rotor is reduced by more than 90%.
-
Key words:
- active magnetic bearing /
- interface contact /
- mode vibration /
- robust control
-
主动磁悬浮轴承（AMB）因其无摩擦、无需润滑、主动可控等优点[1]，可支承转子高速稳定运行，被广泛地应用于旋转机械中. 叶轮作为磁悬浮旋转机械的集成部件，一般通过螺栓连接的方式将其和转子装配，但在工程中陆续发现，增加螺栓连接的预紧力矩，转子和叶轮之间形成的界面接触可能会在转子悬浮时激发转子的高频模态振动，从而导致系统失稳和设备故障，这种现象已经在磁悬浮压缩机[2]和磁悬浮涡轮分子泵[3]中陆续出现，因此，需要研究这种振动的机理并设计控制方法抑制其振动. 由于转子陀螺效应的影响，被激发的模态振动频率随转速明显变化，给振动控制方法研究带来难度，但目前针对此现象的机理和控制策略研究较少，难以预测模态频率的变化并展开相应控制.
磁悬浮轴承具有主动控制的优势，针对界面接触引起的高频振动，一方面可以将其作为干扰，对其进行估计，并针对该扰动进行前馈补偿，但是，常用的前馈补偿如干扰观测器[4]和自抗扰控制器[5]仅适用于低频扰动. 同时，磁悬浮轴承功率放大器较难生成高频电磁力对高频干扰进行抵消，因此，不能用于转子弯曲模态振动控制. 另一方面可以从跨弯曲临界控制的角度抑制模态共振，Tang等[6]采用最优控制从鲁棒性和稳定性的角度提升系统在弯曲临界的阻尼，但并没有考虑参数不确定性的问题. 从在弯曲模态处增加阻尼角度：Jin等[7-8]通过相位补偿提供转子模态频率处的阻尼，从而抑制转子的模态共振；Zheng等[9]在此基础上开展最优阻尼控制，但相位补偿适用于窄带频率，控制范围远小于模态频率变化范围；Roy等[10]提出四元素控制法，基于黏弹性材料耗能减振特性设计控制律，但控制器并联的微分环节会放大高频信号，不利于高频模态振动控制. 从将模态分量从位移信号中分离的角度，Tang等[11]研究适用于挠性转子的模态分离方法，基于弯曲模态振型数据将需要抑制的模态从转子位移中分离，从而抑制模态振动，但该方法对转子模态振型数据依赖程度较高. 控制通道中串入陷波器的方法也较为常用，相关研究[12-13]通过模态实验和仿真确定系统弹性模态频率，假设全转速范围模态频率为恒定值来设计陷波器，在此基础上，提出一种结合转速估计的自适应陷波器 [14]，但当陷波器对该模态振动完全抑制后，转速估计算法难以估计模态振动频率，从而在升速过程中再度激发该模态振动. Mushi等[15]针对压缩机中的气动干扰，基于柔性转子模型设计μ综合控制器，实现对气动扰动的抑制及过临界转速运行. Ran等[16]设计H∞、μ综合控制器，有效抑制了转子的过临界振动.
在界面接触对系统动力学影响机理研究方面，有些研究[17-18]将传统轴承-转子系统中的过盈装配界面接触等效为结构内阻尼，并研究界面接触引入的反对称刚度矩阵对系统稳定性的影响. Liu等[19]和Wu等[20]通过弹簧单元模拟拉杆转子轮盘之间的界面接触，分别研究在平衡力和非线性油膜力激励下界面接触对转子响应的影响，可以发现界面接触会导致系统稳定性下降，但都基于不平衡力、油膜力等激励下. 因此，可以认为界面接触对系统激励起了放大作用. 在磁悬浮轴承-转子系统中，魏彤等[21]将盘与转子之间的过盈配合界面接触等效为旋转方向的弹簧单元，将弹簧单元因转子振动产生的力加入到系统中，并通过实验验证了该假设. 因此，可以把磁悬浮轴承的能量输入等效为激励经过界面接触放大后形成干扰，激发系统的不稳定模态共振. 文献[12]中，激发的模态共振呈现发散状态，升速仿真失稳，无法得到变化的模态频率.
本文通过均布的无质量弹簧单元模拟转子螺栓连接处的界面接触，构建界面接触力扰动并带入磁悬浮轴承-转子系统机电一体化模型. 相比于之前的研究，一是将界面接触的影响考虑为内部扰动，二是考虑界面接触面积随着转子运动状态动态变化，提出时变界面接触模型，得到转子稳态模态共振. 更重要的是，针对振动频率随转速变化带来的不确定性问题，设计考虑频率加性不确定性的H∞ 鲁棒控制器，在实验中实现了振动频率范围内宽频振动抑制效果，保证了系统的全转速稳定运行.
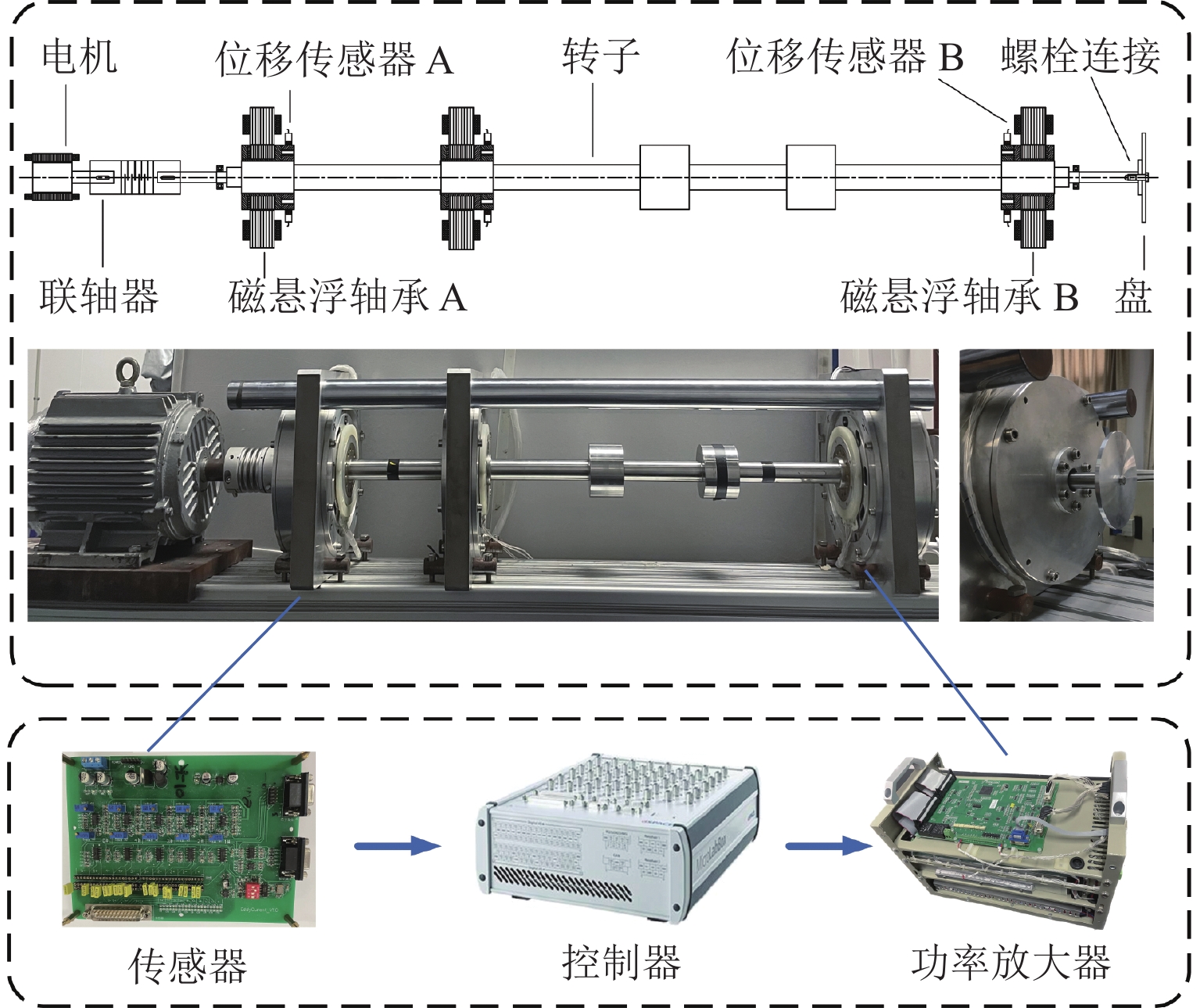
1. 实验台介绍
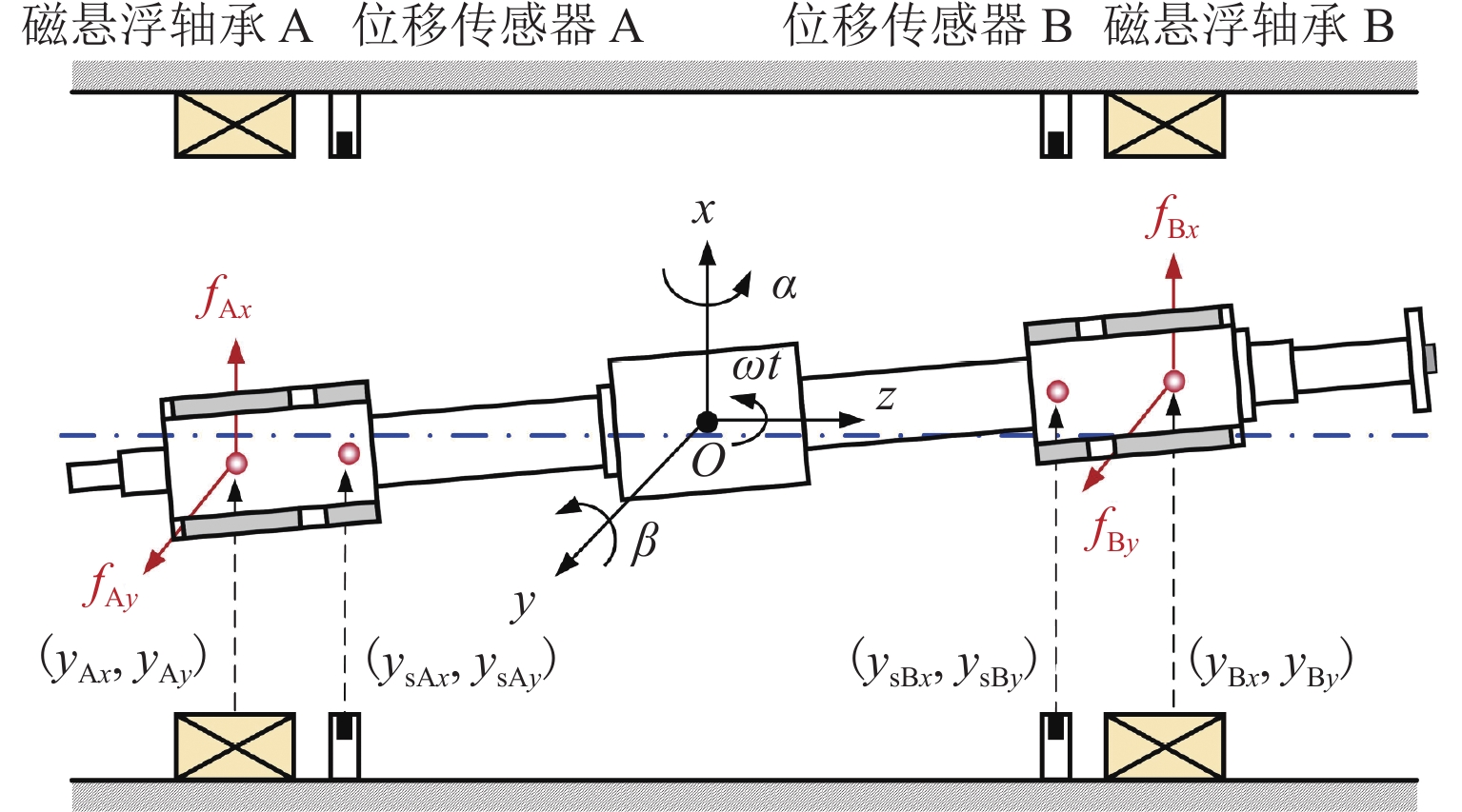
本文采用的磁悬浮轴承转子系统如图1所示，包括机械系统和电控系统. 机械系统中，转子长1.002 m，重约10.35 kg，由2个径向磁悬浮轴承所支承，设计转速高达6000 r/min. 径向磁悬浮轴承的结构参数如表1所示.
表 1 径向磁悬浮轴承结构参数Table 1. Structural parameters of radial magnetic bearing参数 取值 单个磁极匝数 N/匝 75 单个磁极面积 A/m2 4.05 × 10－4 偏置电流 I0/A 2 单边气隙 C0/mm 0.25 对含螺栓连接的转子开展模态试验，得到自由状态下转子的理论和试验弯曲模态频率，如表2所示.
表 2 理论和试验弯曲模态频率Table 2. Theoretical and experimental bending mode frequenciesHz 弯曲模态阶数 理论值 试验值 1 91.26 91.83 2 255.14 257.12 3 526.41 519.53 4 816.02 817.46 5 1046.77 1032.57 2. 磁悬浮轴承-转子系统建模
将磁悬浮轴承-转子系统的建模分成磁悬浮轴承-转子系统建模以及界面接触建模.
2.1 磁悬浮轴承-转子系统模型
磁悬浮轴承-转子系统的建模分为机械系统建模和电控系统建模. 机械系统的建模主要为转子的有限元建模，如图2所示. 图2中：α为绕x轴的转动角度；β为绕y轴的转动角度，后文带下标为对应节点的角度；t为时间；ω为转子旋转角频率； famb =[fAx fAy fBx fBy]，为径向磁悬浮轴承A、B分别在x、y方向产生的电磁力向量；qs=[ysAx ysAy ysBx ysBy]T，为位移传感器A、B测得转子在各位移传感器处的位移；qa=[yAx yAy yBx yBy]T，为磁悬浮轴承处传感器A、B的转子位移向量.
磁悬浮轴承-转子系统的运动方程可以写成
MR¨q+(CR+ωGR)˙q+KRq=TTafamb+TTufu， (1) 式中：MR、CR、GR和KR分别为转子的质量、结构阻尼、陀螺和结构刚度矩阵；Ta为磁悬浮轴承节点的转换矩阵；fu为不平衡质量在x/y方向产生的不平衡力向量；Tu为不平衡质量节点的转换矩阵；q为转子节点的广义坐标向量，q=(qi)T，qi=[xi yi αi βi]，为节点位移向量；xi （yi）、αi （βi）分别为第i个节点的x （y）方向的横向位移及绕x （y）轴的转动自由度.
磁悬浮轴承通过电磁力实现悬浮，其结构如图3所示，磁悬浮轴承A在x方向的线性化电磁力为
fax=μ0AN2I20cos2αC30yAx+μ0AN2I0cosαC20ia_Ax=kxyAx+kiia_Ax, (2) 式中：ia_Ax为线圈控制电流；yAxcos α为磁极与转子之间气隙的实际变化量；μ0为真空磁导率；kx=2.51 × 106 N/m，为位移刚度；ki=338.54 N/A，为电流刚度.
结合式（1）和式（2），可以得到磁悬浮轴承转子系统的状态空间方程为
{qs=[Cr1Cr2]xr,˙xr=[˙q¨q]=[Ar1Ar2Ar3Ar4][q˙q]+[Br1Br2]ia+[0Br3]fu, (3) 式中：Ar1=0，Ar2=I，Ar3=M−1R(kxTaTTa−KR)，Ar4=－M−1R×(CR+ωGR)，Br1=0，Br2=kiM−1RTaT，Br3=M−1RTuT，Cr1=Ts，Ts为传感器检测点的转换矩阵，Cr2=0，ia为控制电流.
电控系统建模中包括位移传感器、功率放大器、控制器的建模，采用比例-积分-微分（PID）控制实现转子的稳定悬浮. ia与由传感器检测的径向转子位移的关系如式（4）所示.
ia=Ga(s)Gc(s)[ur−Gs(s)qs]=(2πfz)kas + (2πfz)(KP+KIs+KDsTDs+1)(ur−ksqs), (4) 式中：Gs(s)、Ga(s)和Gc(s)分别为传感器、功率放大器和控制器的传递函数，ks为传感器增益（ks=20 000），ka为功率放大器增益（ka=0.4），fz为功率放大器截止频率（fz=800 Hz），KP、KI和KD分别为比例增益、积分增益和微分增益，TD为微分时间常数，ur为参考电压向量.
2.2 螺栓连接界面接触模型
如图4（a）所示，通过均布的无质量弹簧单元模拟转子和盘之间的螺栓连接界面接触，弹簧刚度定义为接触刚度，垂直与接触面方向为法向，平行与接触面方向为切向，在法向和切向的接触刚度分别为kf、kq，转子接触面的半径定义为接触半径Rb. 点C为转子接触界面上任意一点，其坐标为（xC, yC, zC）；点D为点C在盘接触界面上的对应点，其坐标为（xD, yD, zD），弹簧单元连接点C和点D；r为点C （D）到圆心m （n）的距离. 如图4（b）所示，转子接触界面中心点m和盘接触界面中心点n均为转子建模节点，在绝对坐标系O-xyz下，点m的广义坐标为（xm,ym,αm,βm），点n的广义坐标为（xn,yn,αn,βn）. 通过坐标变化将绝对坐标系O-xyz变换到随动坐标系m-x3y3z3和n-x3y3z3. 随动坐标系分别以点m和点n为原点，z方向垂直于接触面，O-xy平面与接触面重合. 以随动坐标系m-x3y3z3为例，以点m为坐标原点，xC=rcos θ，yC=rsin θ，进一步通过卡尔丹角坐标变化得到O-xyz下点C和点D的坐标[12].
通过累加每个弹簧单元变形的总能量[19]，可以得到整个界面的能量如式（5）.
E=∬ (5) 式中：Δx=xD−xC，Δy=yD−yC，Δz=zD−zC，Ab为积分区域.
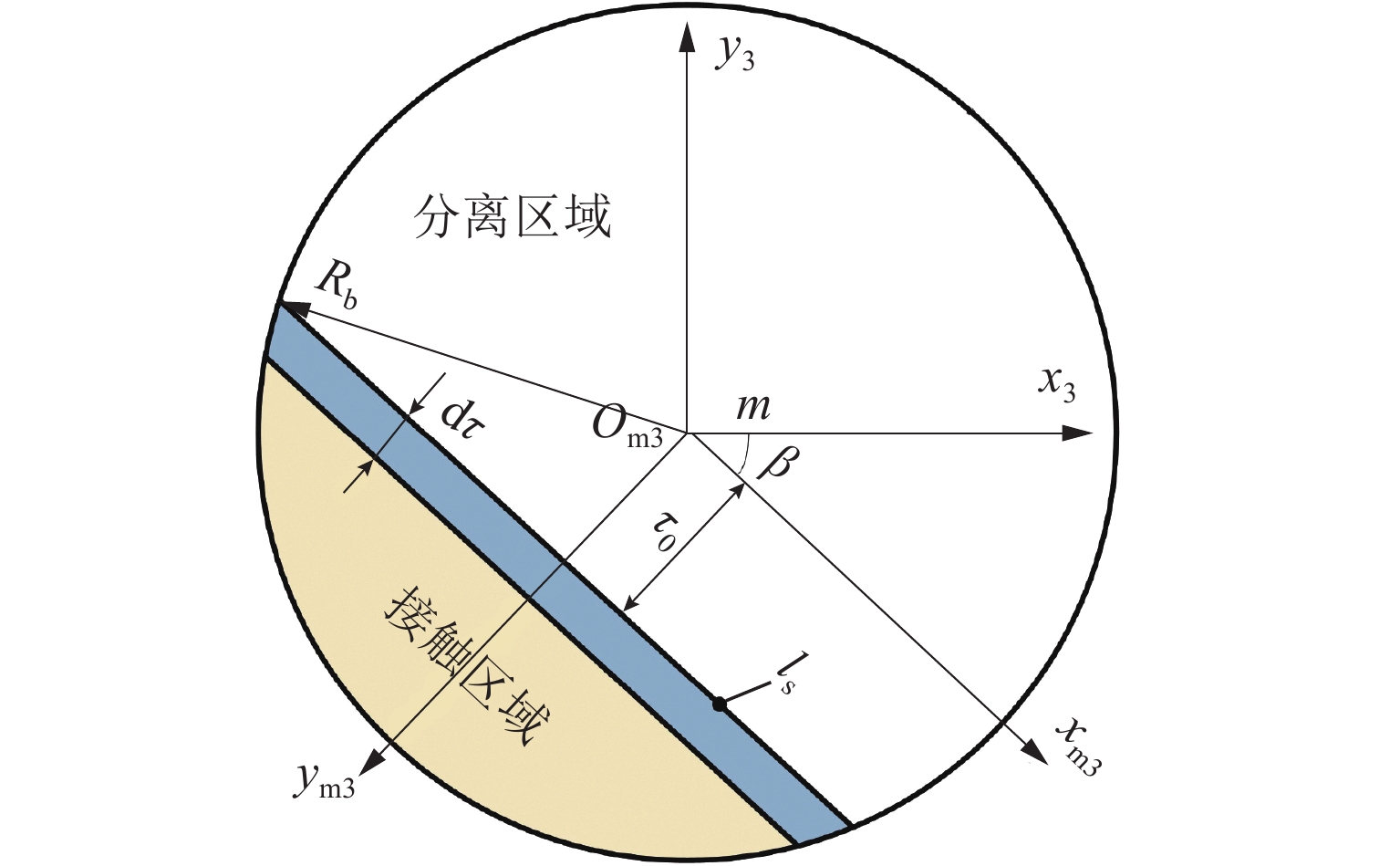
文献[20]中指出，在转子振动时转子和盘的接触界面并非是完全接触的，如图5所示，在接触面上有一条分割线ls，这条分割线将接触界面分为接触区域和分离区域. 界面接触产生的能量随接触区域动态变化. 为求解动态界面力，需要求得分割线的动态方程ax + by + c=0，通过在转子和盘接触面上取任意3点，可以确定这2个界面的空间方程，从而确定该时刻a、b、c的值. 如图5所示，从点m到分割线ls的距离为τ0=|c|/（a2 + b2）0.5，通过取图中的微分单元并进行积分可以得到. 界面振动能量中的时变积分量为
\left\{ {\begin{array}{*{20}{l}} {\displaystyle\iint {{\mathrm{d}}{A_{\text{b}}} = \displaystyle\int_{\tau_0} ^{{R_{\mathrm{b}}}} {2R_{\mathrm{b}}\sqrt {1 - {{\left( {\dfrac{y_{\mathrm{m3}} }{{{R_{\mathrm{b}}}}}} \right)}^2}} } }{\mathrm{d}}y_{\mathrm{m3}} }, \\ \displaystyle\iint {{y^2}{\mathrm{d}}{A_{\text{b}}} ={\cos ^2}\beta \displaystyle\int_{\tau_0} ^{{R_{\mathrm{b}}}} {2{y_{\mathrm{m3}} ^2}\sqrt {{R_{\mathrm{b}}}^2 - {y_{\mathrm{m3}} ^2}} } }{\mathrm{d}}y_{\mathrm{m3}}+ \\ \quad {\sin ^2}\beta\displaystyle\int_{\tau_0} ^{{R_{\mathrm{b}}}} {4{{\left( {{R_{\mathrm{b}}}^2 - {y_{\mathrm{m3}} ^2}} \right)}^{1.5}}} {\mathrm{d}}y_{\mathrm{m3}}, \\ \displaystyle\iint {{x^2}{\mathrm{d}}{A_{\text{b}}} = {\sin ^2}\beta \displaystyle\int_{\tau_0} ^{{R_{\mathrm{b}}}} {2{y_{\mathrm{m3}} ^2}\sqrt {{R_{\mathrm{b}}}^2 - {y_{\mathrm{m3}} ^2}} } }{\mathrm{d}}y_{\mathrm{m3}}+ \\ \quad \displaystyle\int_{\tau_0} ^{{R_{\mathrm{b}}}} {4{{\left( {{R_{\mathrm{b}}}^2 - {y_{\mathrm{m3}} ^2}} \right)}^{1.5}}} {\mathrm{d}}y_{\mathrm{m3}} {\cos ^2}\beta. \end{array} } \right. (6) 对能量求导得到作用在界面的力Fc为
\begin{split} & {{\boldsymbol{F}}_{\mathrm{c}}} = \left[ {\begin{array}{*{20}{c}} {{k_{\mathrm{q}}}\iint {{\mathrm{d}}{A_{\text{b}}} \Delta {x_{mn}}}}&{{k_{\mathrm{q}}}\iint {{\mathrm{d}}{A_{\text{b}}} \Delta {y_{mn}}}} \end{array}} \right. \\ &\;{\left. {\begin{array}{*{20}{c}} {{k_{\mathrm{f}}}\iint {{y^2}}{\mathrm{d}}{A_{\text{b}}} \Delta {\alpha _{mn}}}&{{k_{\mathrm{f}}}\iint {{x^2}}{\mathrm{d}}{A_{\text{b}}} \Delta {\beta _{mn}}} \end{array}} \right]^{\mathrm{T}}} , \end{split} (7) 式中：Δxmn=xm−xn，Δymn=ym−yn，Δαmn=αm−αn，Δβmn= βm−βn.
2.3 整体系统模型动力学特性分析
文献[22]中，将螺栓连接界面接触的影响等效为作用在磁悬浮轴承-转子系统上的力扰动. 通过将机械系统、控制系统、界面接触模型相结合，得到系统闭环数学模型，如式（8）.
\begin{split} &{{\boldsymbol{M}}_{\mathrm{R}}}\ddot {\boldsymbol{q}} + \left( {{{\boldsymbol{C}}_{\mathrm{R}}} + \omega {{\boldsymbol{G}}_{\mathrm{R}}}} \right)\dot {\boldsymbol{q}} + {{\boldsymbol{K}}_{\mathrm{R}}}{\boldsymbol{q}} =\\ &\quad{k_{\mathrm{x}}}{\boldsymbol{T}}_{\mathrm{a}}^{\mathrm{T}}{{\boldsymbol{T}}_{\mathrm{a}}}{\boldsymbol{q}} + {k_{\mathrm{i}}}{\boldsymbol{T}}_{\mathrm{a}}^{\mathrm{T}}{i_{\mathrm{a}}} + {\boldsymbol{T}}_{\mathrm{u}}^{\mathrm{T}}{{\boldsymbol{f}}_{\mathrm{u}}} + {\boldsymbol{T}}_{\mathrm{c}}^{\mathrm{T}}{{\boldsymbol{F}}_{\mathrm{c}}} ,\\ \end{split} (8) 式中：Tc为界面接触点转换矩阵.
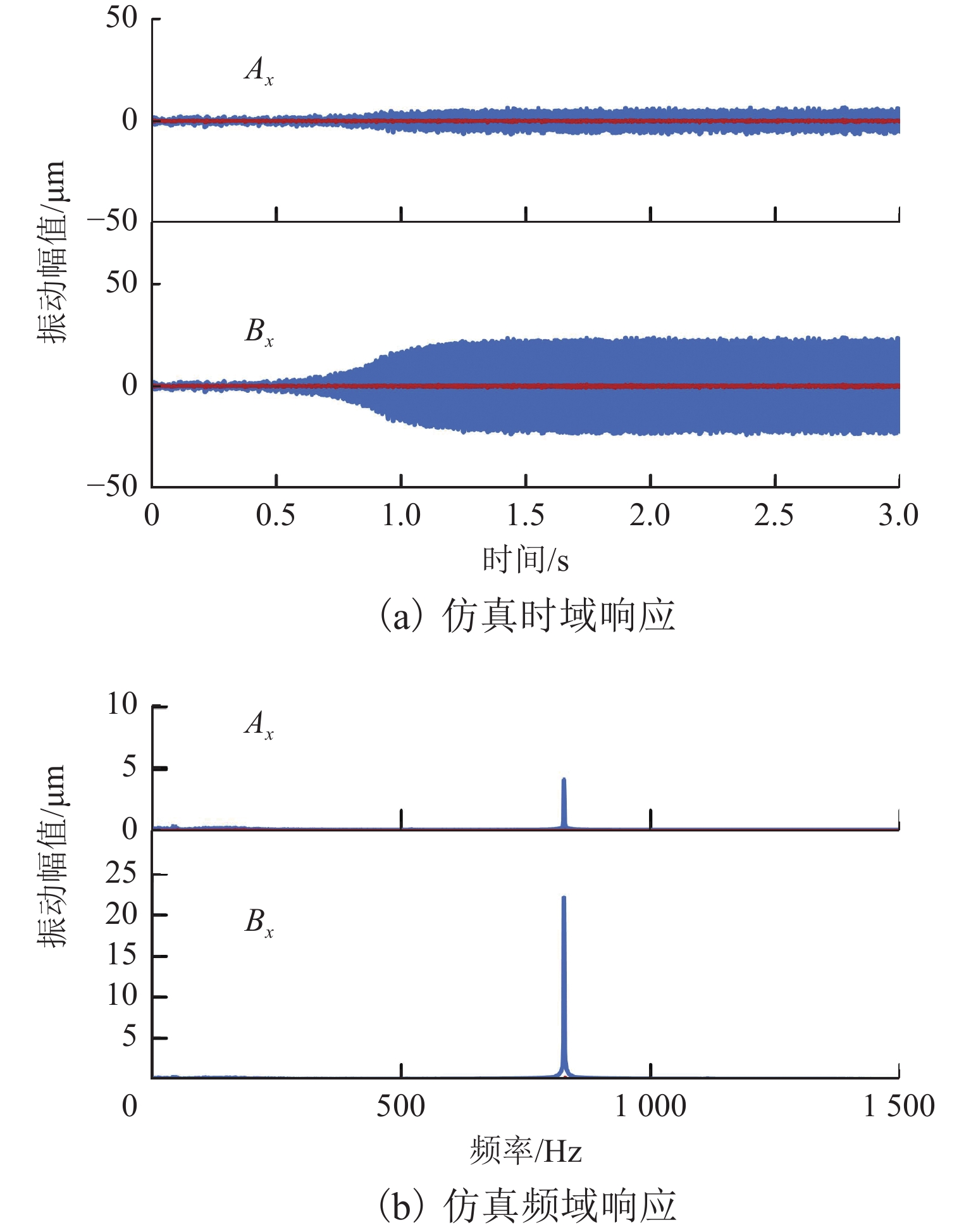
基于该模型进行数值仿真，得到转子在静态悬浮下的响应，如图6所示. 图中：Ax（Bx）为磁悬浮轴承A（B）处转子在x方向的位移. 可以看到：当转子和盘之间不存在界面接触时，转子在PID控制下可以稳定悬浮，从频域上看无明显的振动频率；当考虑转子和盘之间的界面接触，转子从初始的稳定悬浮进入到振幅发散并经过一定的时间进入到稳态模态振动，磁悬浮轴承B处（靠近界面接触端）的振幅大于磁悬浮轴承A处的振幅，并且转子的振动频率为转子的四阶弯曲模态频率.
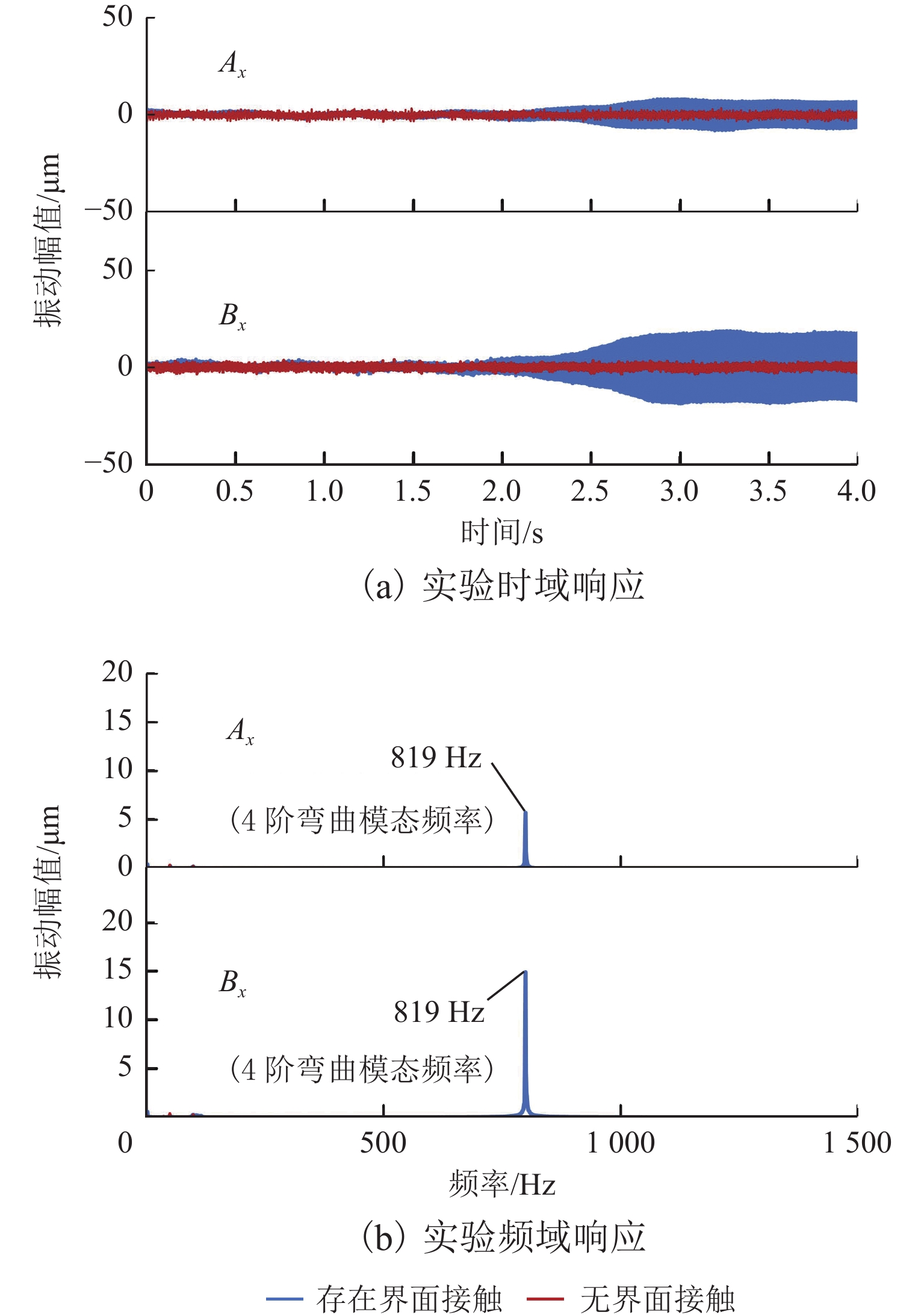
图 6 磁悬浮轴承转子系统静态悬浮仿真响应Figure 6. Static levitation simulation response of magnetic bearing-rotor system为模拟无界面接触状态，在实验中未拧紧盘和转子之间的螺栓连接，该工况下，转子在PID控制下可以稳定悬浮，从频域上看无明显的振动频率；采用2 N•m的力矩拧紧螺栓，转子振幅逐渐变大，最终进入稳态模态振动. 如图7所示，实验时域和频域结果验证了模型的准确性，并揭示螺栓连接形成的界面接触是导致转子静态悬浮下模态振动的主要原因. 仿真和实验中PID控制器参数：KP=1.7，KI=1，KD=0.0006，TD=0.0001，计算步长（采样频率）为10 kHz.
图 7 磁悬浮轴承转子系统静态悬浮实验响应Figure 7. Static levitation experiment response of magnetic bearing-rotor system通过实验分析PID控制器参数对系统响应的影响规律，KP和KI参数对系统稳定性影响较小. 增加KD参数，控制器中的微分单元提升了系统的高频增益，放大了模态振动的影响，减小KD参数，闭环系统的稳定性也相应减小，降低系统面对干扰时的鲁棒性. 因此，反馈控制中仅仅依靠PID控制器是无法抑制由界面接触引起的模态振动.
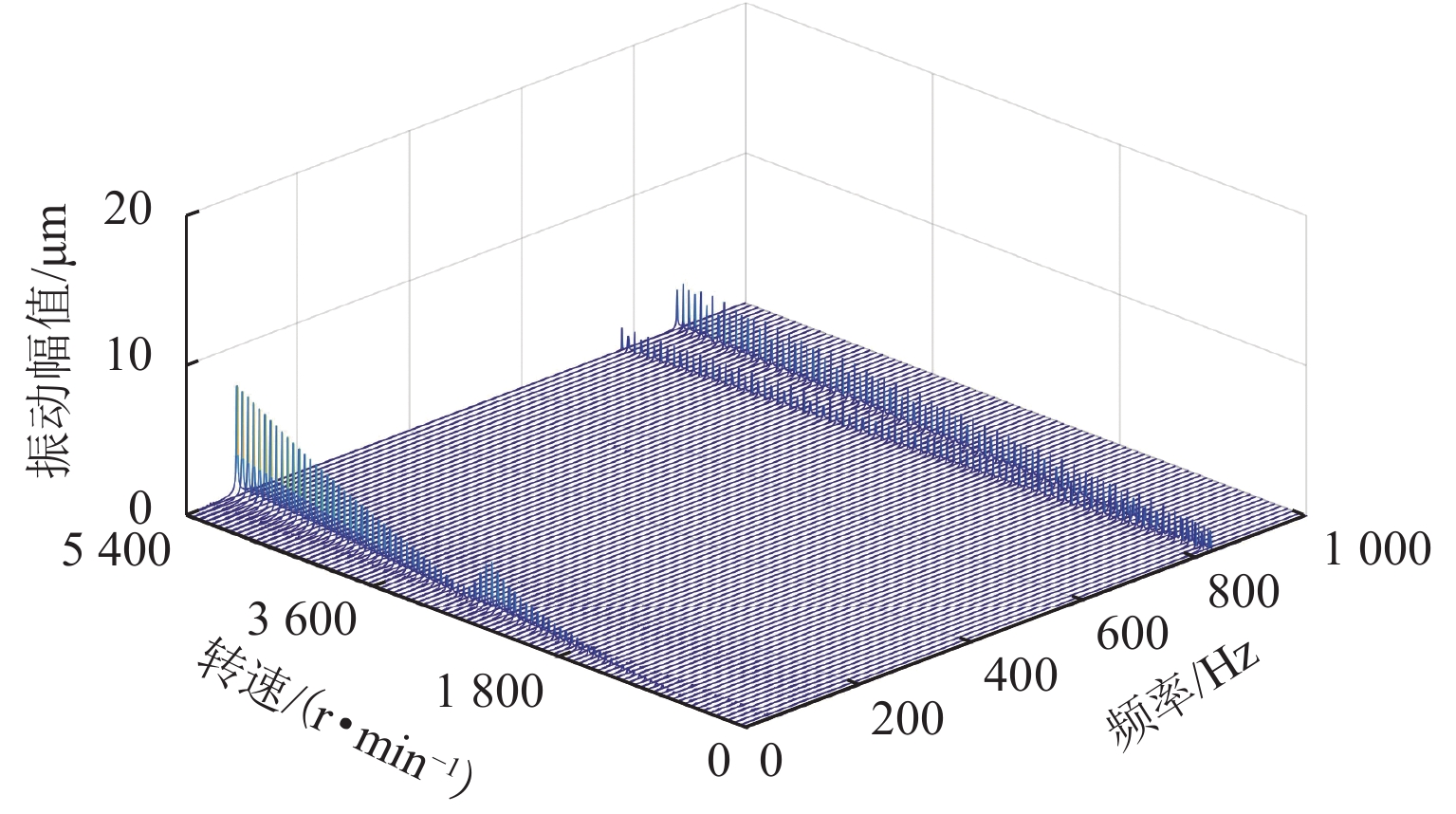
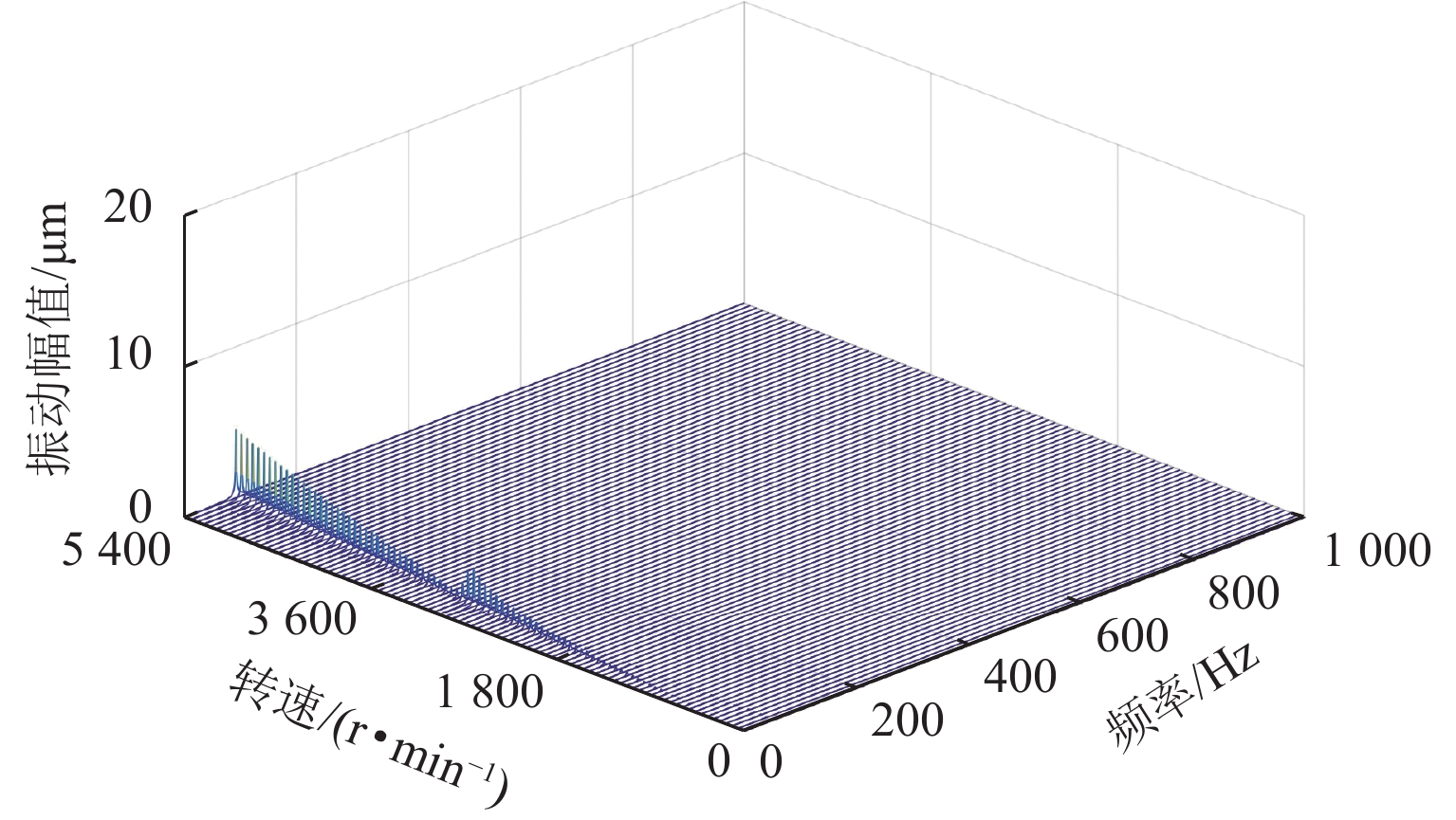
转子升速仿真结果如图8所示. 由图可知：界面接触激发的模态振动频率随着转速发生变化，分别触发转子的四阶弯曲模态正涡动和反涡动；当转速升至90 Hz时，正涡动频率和反涡动频率相差40 Hz. 综上，在转子升速过程中振动频率存在不确定性，因此，需要针对这种不确定性开展振动控制方法研究.
3. 控制器设计
控制器的设计需要考虑振动频率不确定性. H∞ 控制作为鲁棒控制，对磁悬浮轴承-转子系统的模型参数摄动及其他不确定性均有较好的鲁棒性.
3.1 鲁棒控制理论及控制结构描述
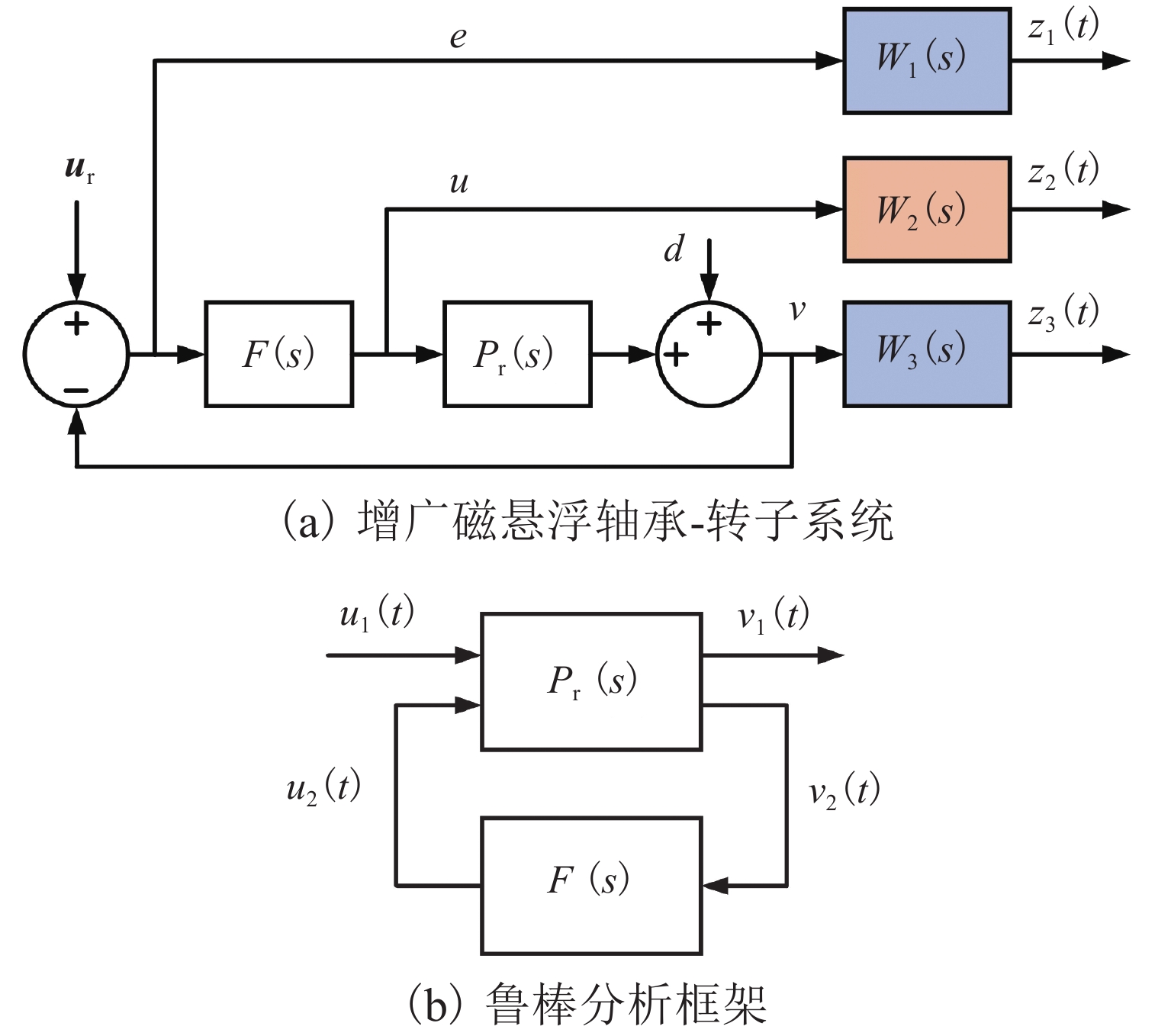
在H∞ 控制器的设计过程中，其目的是寻找一个稳定的控制器，能够实现输入信号到输出信号的最小化，或满足一定的指标，通常都是将设计模型转化为一般混合灵敏度问题进行求解[16]. 混合灵敏度H∞ 鲁棒控制算法可以同时考虑干扰和建模不确定性，且控制器性能通过选取合适的加权函数实现平衡. 其加权控制结构如图9所示. 图9中：F(s)为控制系统函数；Pr(s)为被控对象函数，如式(9)；ur、e、u、d、v分别为参考信号、偏差信号、控制信号、干扰信号、输出信号；W1(s)、W3(s)分别为系统的灵敏度、补灵敏度加权函数；W2(s)为加性不确定性而引入系统的加权函数；z1(t)、z2(t)和z3(t)为观测信号；u1(t)/u2(t)为外部输入；v1(t)/v2(t)为外部输出. W1(s)被用于设计灵敏度函数S(s)的边界，从而减小稳态误差和保证对低频扰动的抗干扰能力. W3(s)被用于设计补灵敏度函数T(s)的边界，T(s)的主要干扰为模型不确定性带来的，并且主要体现在高频段. W2(s)为对Q(s)的权函数，Q(s)为参考信号R(s)到控制电压信号u的闭环传递函数，其幅值直接影响控制量大小及系统控制带宽.
\begin{split}& {{\boldsymbol{P}}_{\text{r}}}\left( s \right) = \left[ {\begin{array}{*{20}{c}} {{{\boldsymbol{P}}_{{\mathrm{r}}11}}\left( s \right)}&{{{\boldsymbol{P}}_{{\mathrm{r}}12}}\left( s \right)} \\ {{{\boldsymbol{P}}_{{\mathrm{r}}21}}\left( s \right)}&{{{\boldsymbol{P}}_{{\mathrm{r}}22}}\left( s \right)} \end{array}} \right] =\\ &\quad\left[ {\begin{array}{*{20}{c}} {{{\boldsymbol{A}}_{\mathrm{P}}}}&{{{\boldsymbol{B}}_{{\mathrm{P}}1}}}&{{{\boldsymbol{B}}_{{\mathrm{P}}2}}} \\ {{{\boldsymbol{C}}_{{\mathrm{P}}1}}}&{{{\boldsymbol{D}}_{{\mathrm{P}}11}}}&{{{\boldsymbol{D}}_{{\mathrm{P}}12}}} \\ {{{\boldsymbol{C}}_{{\mathrm{P}}2}}}&{{{\boldsymbol{D}}_{{\mathrm{P}}21}}}&{{{\boldsymbol{D}}_{{\mathrm{P}}22}}} \end{array}} \right].\end{split} (9) 相应的增广状态方程描述为
\left\{\begin{gathered} \dot {\boldsymbol{X}}\left( t \right) = {{\boldsymbol{A}}_{\mathrm{P}}} {\boldsymbol{X}}_{\mathrm{r}} + \left[ {\begin{array}{*{20}{c}} {{{\boldsymbol{B}}_{{\mathrm{P}}1}}}&{{{\boldsymbol{B}}_{{\mathrm{P}}2}}} \end{array}} \right] \left[ {\begin{array}{*{20}{c}} {{u_1}(t)} \\ {{u_2}(t)} \end{array}} \right], \\ \left[ {\begin{array}{*{20}{c}} {{y_1}\left( t \right)} \\ {{y_2}\left( t \right)} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} {{{\boldsymbol{C}}_{{\mathrm{P}}1}}} \\ {{{\boldsymbol{C}}_{{\mathrm{P}}2}}} \end{array}} \right] {\boldsymbol{X}}_{\mathrm{r}} + \left[ {\begin{array}{*{20}{c}} {{{\boldsymbol{D}}_{{\mathrm{P}}11}}}&{{{\boldsymbol{D}}_{{\mathrm{P}}12}}} \\ {{{\boldsymbol{D}}_{{\mathrm{P}}21}}}&{{{\boldsymbol{D}}_{{\mathrm{P}}22}}} \end{array}} \right] \left[ {\begin{array}{*{20}{c}} {{u_1}(t)} \\ {{u_2}(t)} \end{array}} \right]. \\ \end{gathered} \right. (10) 由外部输入u1(t)到外部输出y1(t)的闭环系统传递函数可以写成
{{\boldsymbol{F}}_{\mathrm{l}}}({{\boldsymbol{P}}_{\mathrm{r}}},F) = {{\boldsymbol{P}}_{{\mathrm{r}}11}} + {{\boldsymbol{P}}_{{\mathrm{r}}12}}{\left[ {I - {{\boldsymbol{P}}_{{\mathrm{r}}22}}F} \right]^{ - 1}}F{{\boldsymbol{P}}_{{\mathrm{r}}21}} . (11) 式（9）中ur到z1(t)、z2(t)和z3(t)的传递函数分别为W1S、W2Q、W3T. 为使闭环系统稳定，H∞ 控制器在设计过程中需要令以上3个传递函数的H∞ 范数小于1，如式（12）.
\left\| {\boldsymbol{F}}_{\mathrm{l}}({\boldsymbol{P}}_{\text{r}},F) \right\| _{\infty}= \left\| \begin{array}{*{20}{c}}W_1S \\ W_2Q \\ W_3T\end{array} \right\| _{\infty} < 1. (12) H∞ 控制器设计的关键是3个加权函数和具体的被控对象. 加权函数的选择有以下3点原则：
1） W1(s)通常为一个高增益的带积分特性的低通滤波器，并且与灵敏度函数满足以下要求：
\sigma\left(S({\mathrm{j}}\omega)\right)\leqslant\sigma\left(W_1({\mathrm{j}}\omega)^{-1}\right), (13) 式中：σ(•)为传递函数的奇异值.
2） W2(s)通常被设计为一个小的正数，合理选择W2(s)的大小可以限制控制量u的大小，防止控制系统出现严重的控制电压饱和现象.
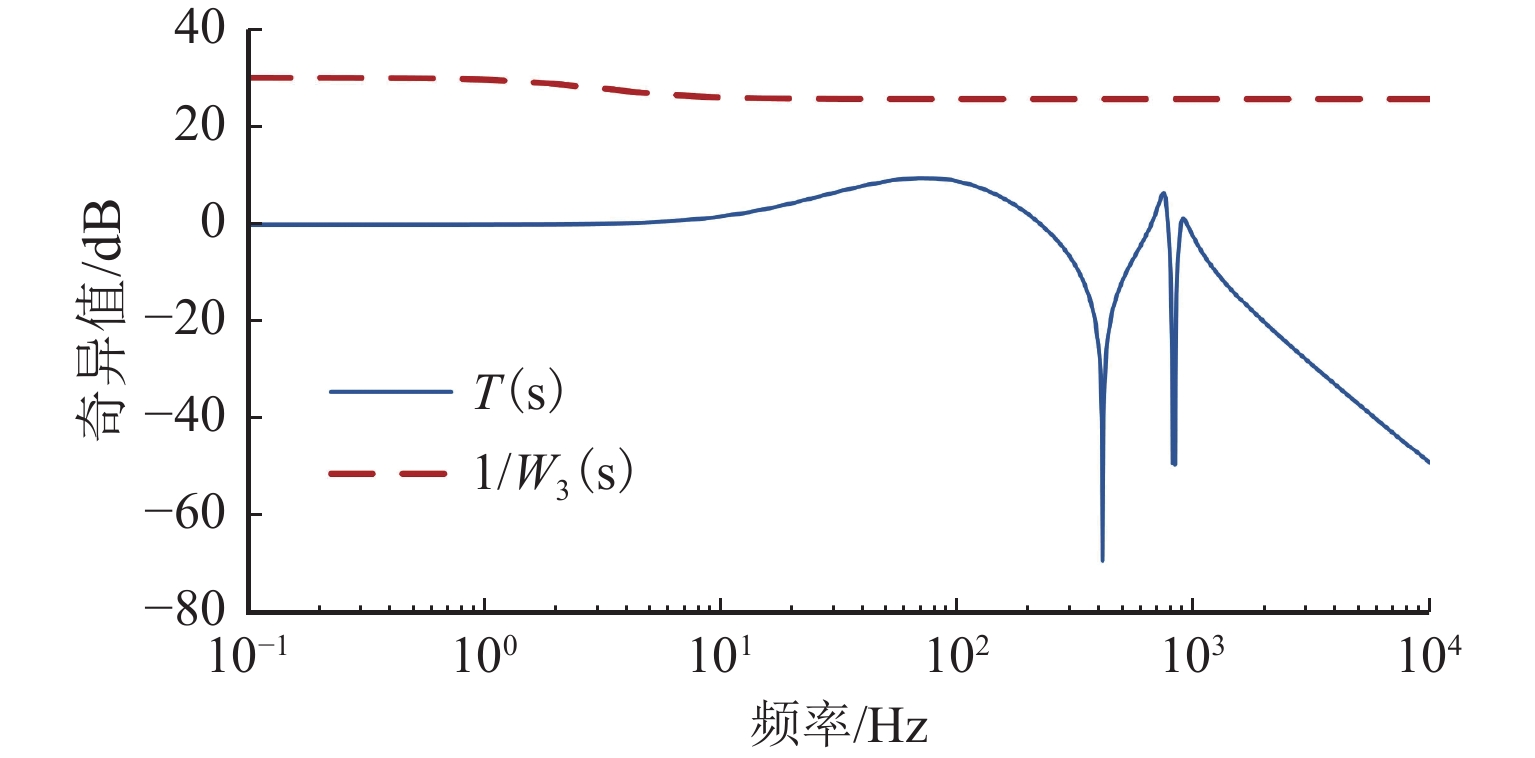
3） W3(s)通常具有高通特性，需要保证W1(s)的截至频率要充分低于W3(s)的截止频率，与补灵敏度函数满足式（14）要求.
\sigma\left(T({\mathrm{j}}\omega)\right)\leqslant\sigma\left(W_3({\mathrm{j}}\omega)^{-1}\right). (14) 基于上述原则，最终加权函数如式（15）所示. 加权函数的奇异值如图10、11所示.
\left\{ \begin{array}{*{20}{l}}W_1\left(s\right)=\dfrac{200}{s+0.5}, \\ W_2\left(s\right)=2.5\times10^{-8},\\ W_3\left(s\right)=\dfrac{0.5s+1.5}{2s+50}.\end{array}\right. (15) 3.2 被控对象构建
在考虑接触界面的磁悬浮轴承转子系统中存在高频模态振动，为了后续控制器的设计，需要建立被控对象函数Pr(s). 被控对象直接影响了后续设计控制器的控制效果.
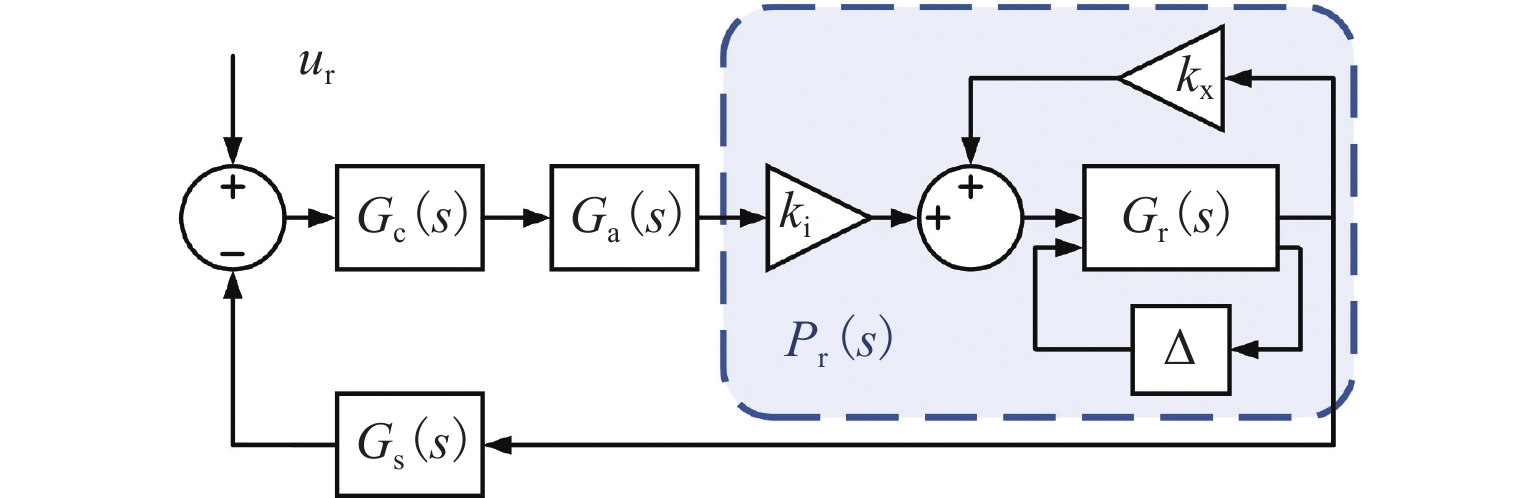
考虑弯曲模态的磁悬浮轴承-转子系统等效框图如图12所示. 图中，Gr(s)为保证传递函数. 被控对象的传递函数阶数最低，同时表达出考虑界面接触的系统模态振动特性，因此，传递函数表达式可以等效为等效刚性模型与四阶弯曲模态的叠加，如式（16）.
{G_{\mathrm{r}}}\left( s \right) = \frac{1}{{{m_{\mathrm{g}}}{s^2}}} + \frac{{{t_{\mathrm{f}}}}}{{{m_{\mathrm{f}}}{s^2} + {d_{\mathrm{f}}}s + {m_{\mathrm{f}}}\omega _f^2}} , (16) 式中：mg为刚体模态质量，mf、df、ωf和tf分别为转子的四阶弯曲模态的模态质量、模态阻尼、模态频率和振型.
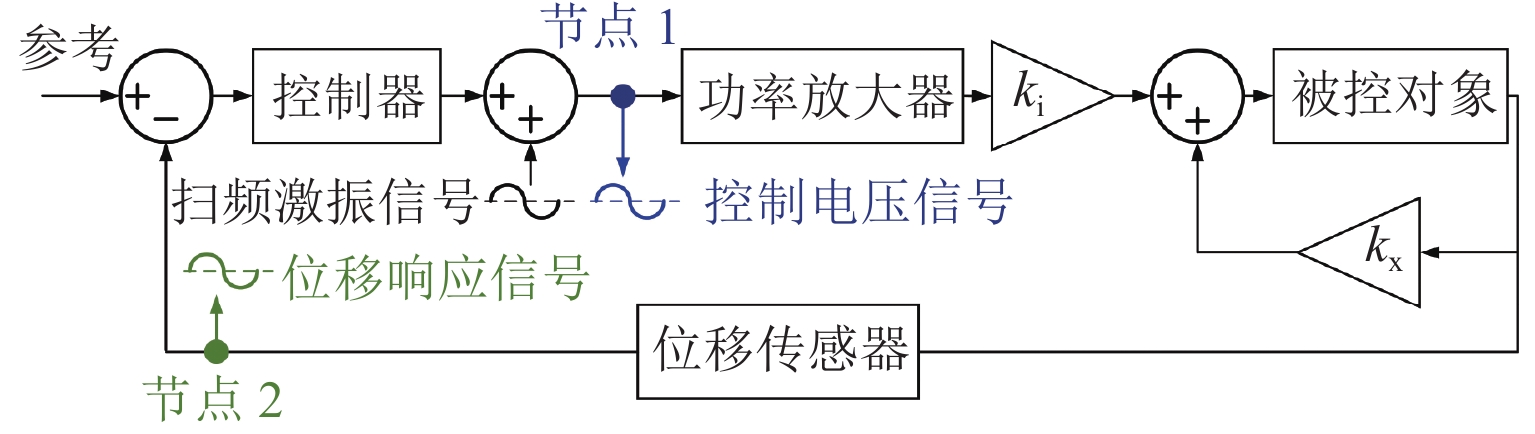
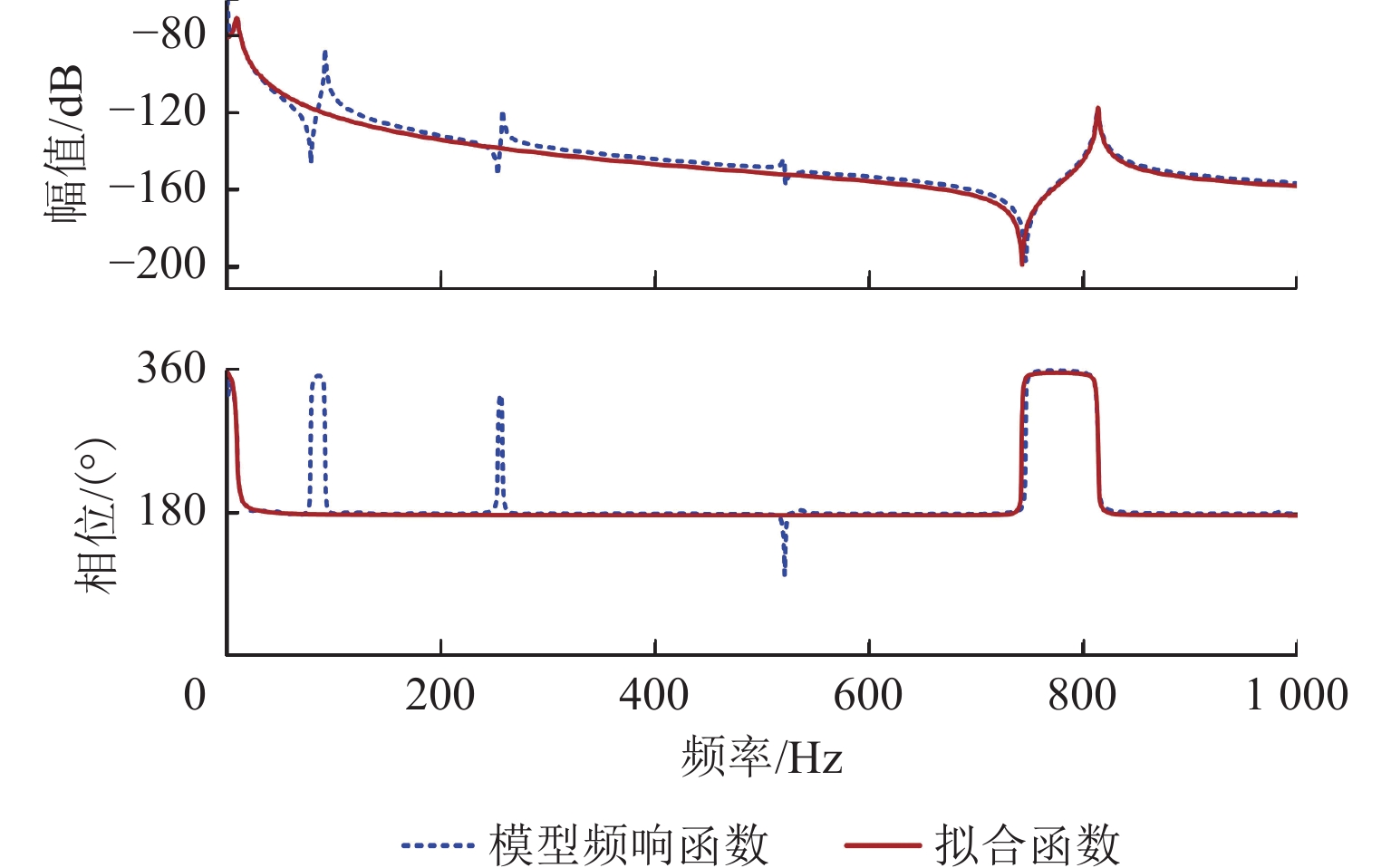
通过对磁悬浮轴承-转子系统开展闭环扫频试验，如图13所示. 节点1为控制电压信号，当转子处于静态悬浮时在控制器输出信号之后加入0~1000 Hz的正弦扫频激励. 节点2为传感器信号检测点，通过位移传感器将转子位移信号转换为电压信号并进行采集，得到从控制电压信号到转子位移电压信号的开环传递函数频率特性，除去功放增益、电流刚度、传感器增益，得到被控对象的频响特性.
图 13 被控对象扫频试验原理框图Figure 13. Sweep frequency experiment principle for controlled object采用式（14）传递函数进行拟合，试验频响和拟合频响如图14所示，模态参数：mg =1，mf=1，df =0.01，ωf =819 × 2π，tf =0.9977.
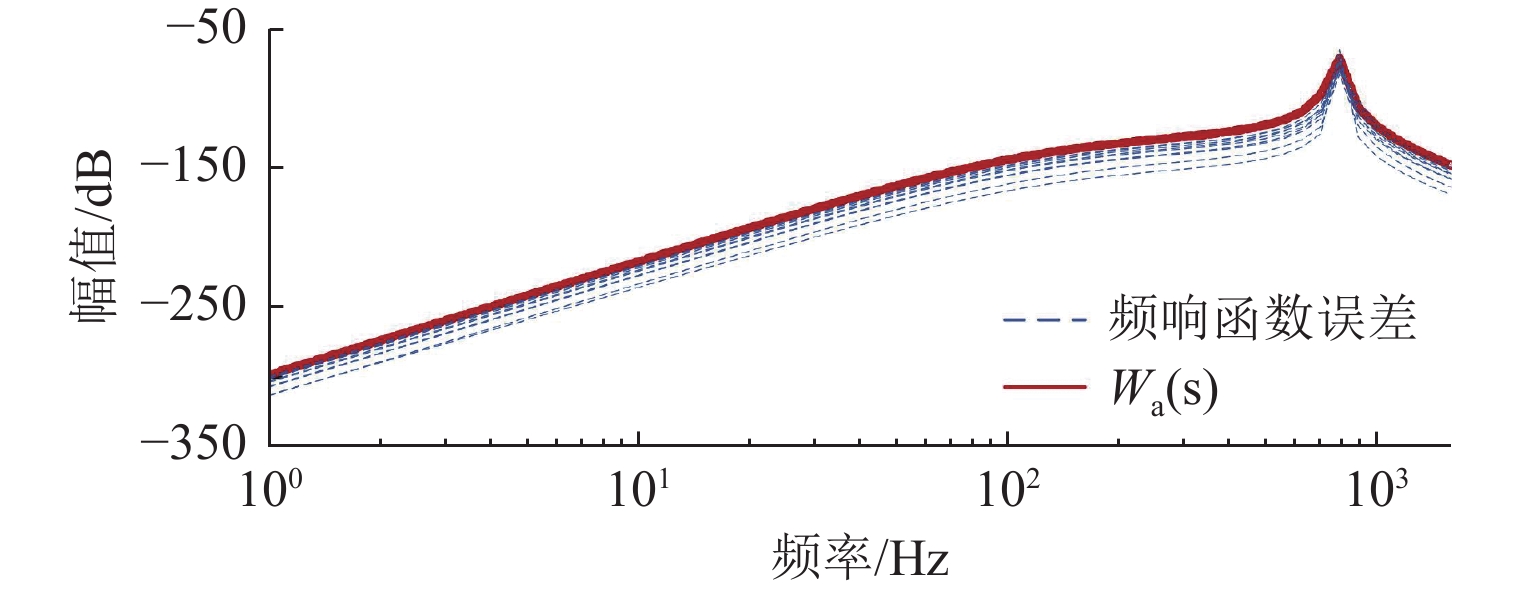
根据动力学分析结果，当转子升至90 Hz时，振动频率相较静态悬浮时的振动频率存在 ±3%以内的摄动，因此，需要建立考虑加性不确定性的转子模型Gra(s)，如式（17）所示.
{G_{{\mathrm{ra}}}}\left( s \right) = {G_{{\mathrm{rr}}}}\left( s \right) + {\varDelta _{\mathrm{a}}}\left( s \right){W_{\mathrm{a}}}\left( s \right)， (17) 式中：|{\varDelta _{\mathrm{a}}}(s) | 为比例增益，|{\varDelta _{\mathrm{a}}} (s)|≤ 1；Grr(s)为ωf =819 × 2π rad/s时的被控对象传递函数；Wa(s)为不确定性的上边界是ωf在±3%摄动范围内取值时频响函数误差Gr(s)−Grr(s)的最大值.
如图15所示，通过频响函数拟合，得到其具体的传递函数.
如图12所示，在Gra(s)的基础上考虑磁悬浮轴承的电流刚度ki和位移刚度kx，可得传递函数Pr(s)如式（18）.
{P_{\mathrm{r}}}\left( s \right) = \frac{{{k_{\mathrm{i}}}{G_{{\mathrm{ra}}}}\left( s \right)}}{{1 - {k_{\mathrm{x}}}{G_{{\mathrm{ra}}}}\left( s \right)}} . (18) 3.3 控制器设计
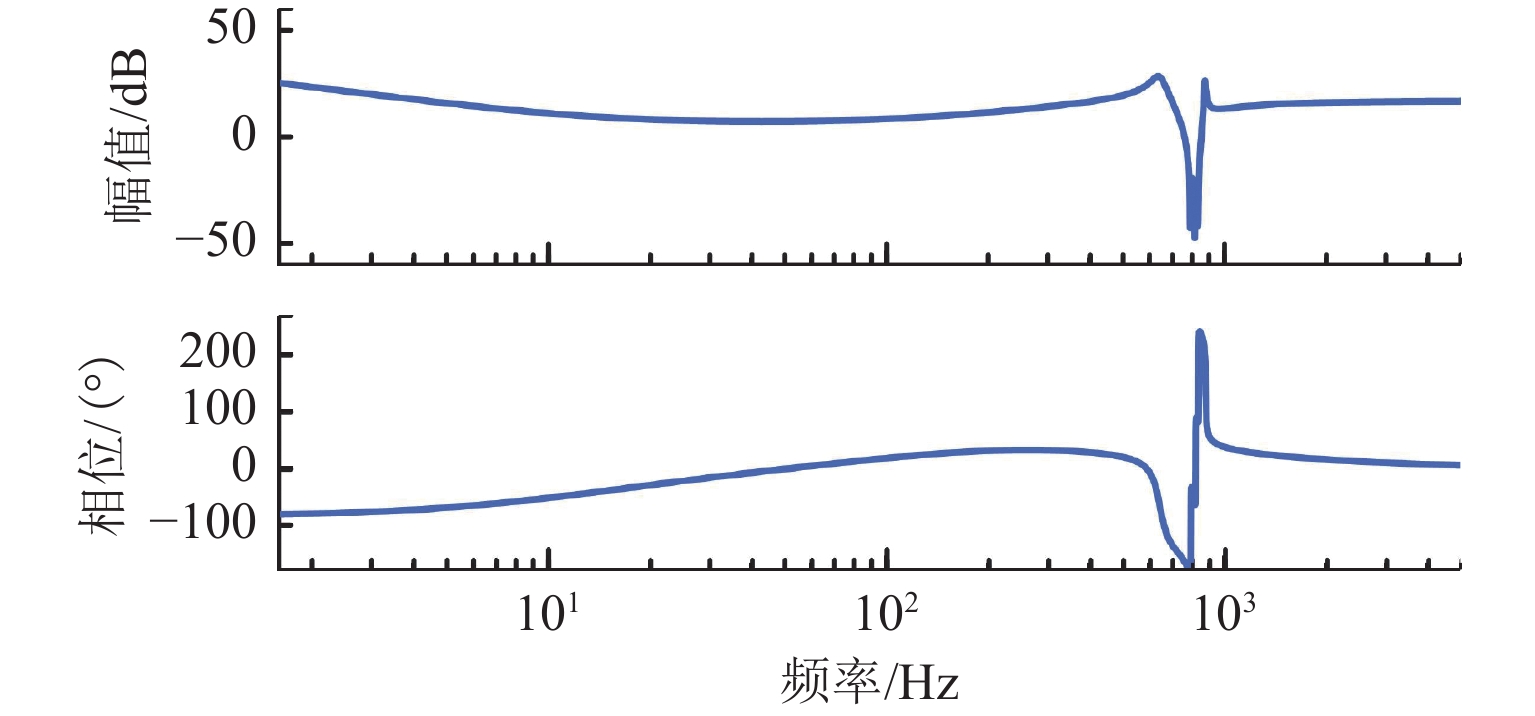
基于式（12）设计所需要的H∞ 控制器，最终求得控制器频率特性如图16所示.
由图16可以看出：在低频段，H∞ 控制器具有明显的积分效应；在中频段可以实现类似于PD控制器的频响特性，在四阶弯曲模态频率范围内，产生类似于宽频带阻滤波器的频响特性来抑制磁悬浮轴承转子系统的弯曲模态振动.
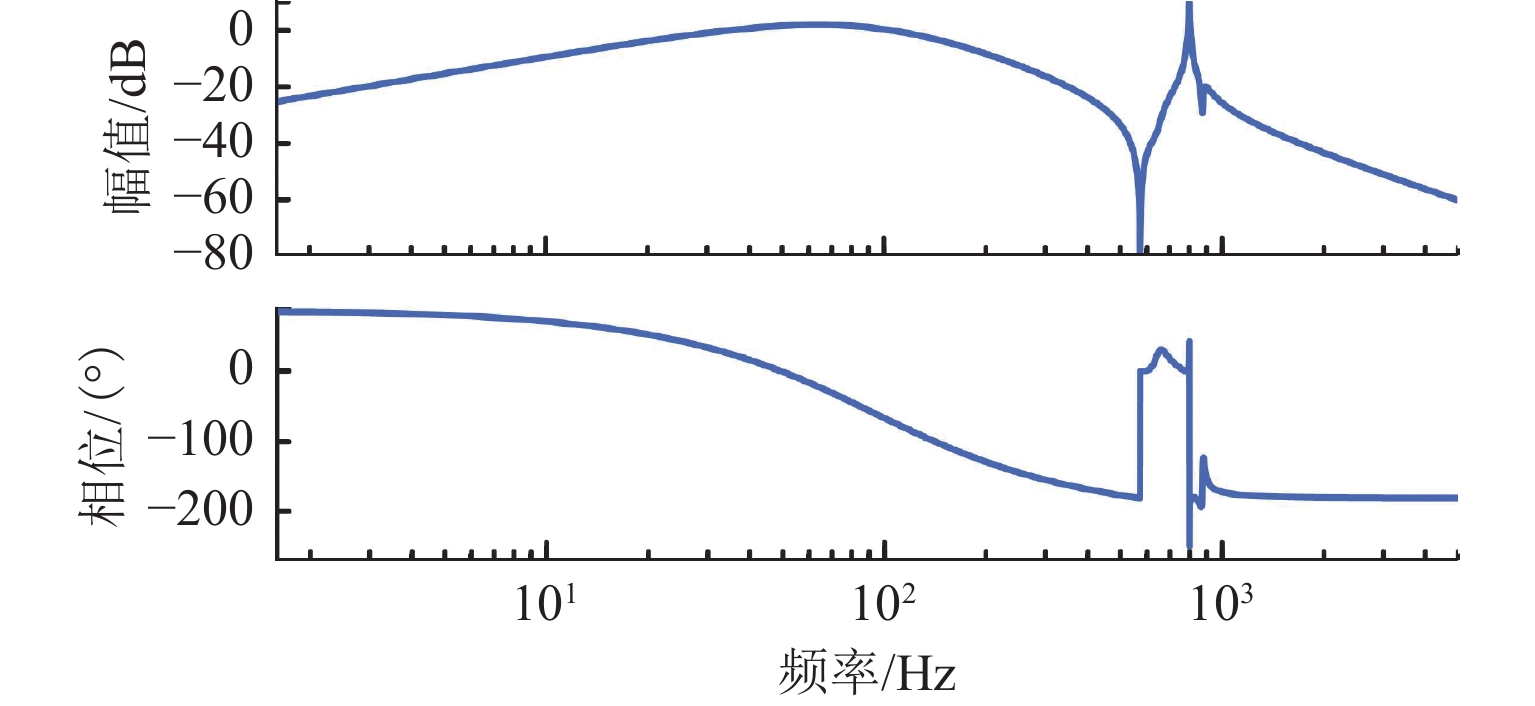
系统的动态柔度频响曲线如图17所示. 可以看出，控制器在四阶弯曲模态频率范围内提供一定的额外阻尼，提高了磁悬浮轴承对转子第四阶弯曲模态振动的抑制能力.
4. 数值仿真与实验验证
4.1 数值仿真结果
基于第2节中建立的磁悬浮轴承-转子系统机电模型，将第3节设计的H∞ 鲁棒控制器在磁悬浮轴承转子系统模型中进行数值仿真验证. 仿真中PID控制参数和计算步长并未改变.
在转子静态悬浮状态下，验证H∞ 鲁棒控制器的性能. 采用H∞ 鲁棒控制器时系统下的转子仿真响应如图18所示. 可以发现，在静态悬浮状态下该控制器能够很好地抑制转子的四阶弯曲模态振动，在时域响应中，磁悬浮轴承处的振动幅值明显减小，且从频域响应中四阶弯曲模态频率已经消失.
为验证该鲁棒控制器在升速后振动频率变化时的振动抑制效果，开展考虑界面接触的转子升速仿真. 采用H∞ 鲁棒控制器时系统下的转子升速响应如图19所示. 可以发现，在升速范围内该控制器能够很好地抑制转子的四阶弯曲模态振动，在时域响应中磁悬浮轴承处的振动幅值明显减小，而从频域响应中四阶弯曲模态频率已经消失.
4.2 实验验证结果
基于第1节所示的磁悬浮轴承-转子系统实验台，将第3节设计的H∞ 鲁棒控制器在磁悬浮轴承转子系统中进行实验验证. 实验中PID控制参数和采样频率并未改变.
在转子静态悬浮状态下，验证H∞ 鲁棒控制器的性能. 采用H∞ 鲁棒控制器时系统下的转子实验响应如图20所示. 可以发现，在静态悬浮状态下该控制器能够很好地抑制转子的四阶弯曲模态振动，在时域响应中磁悬浮轴承处的振动幅值明显减小，且从频域响应中四阶弯曲模态频率已经消失.
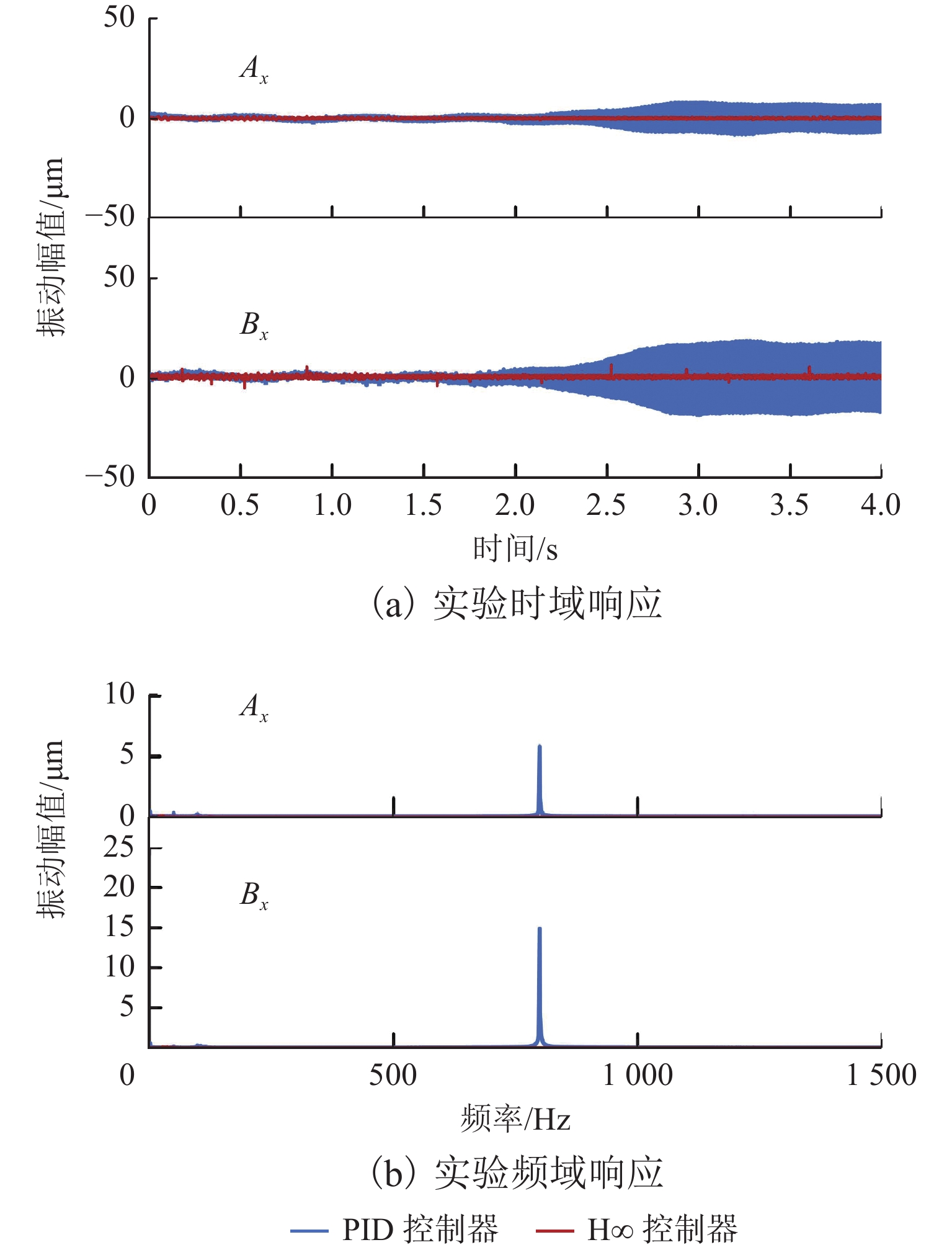
为验证该鲁棒控制器在升速后振动频率变化时的振动抑制效果，开展考虑界面接触的转子升速实验. 图21（a）所示为采用PID控制的磁悬浮轴承转子系统0~6000 r/min升速响应，界面接触激发的四阶弯曲模态振动频率随转速发生变化，发生正向和反向涡动. 图21（b）中，采用所设计的H∞ 鲁棒控制器在升速范围内能够很好地抑制转子的四阶弯曲模态振动，在时域响应中，磁悬浮轴承处的振动幅值明显减小，而从频域响应中四阶弯曲模态分量被大幅削减.
5. 结 论
磁悬浮轴承-转子系统中，由于转子装配界面接触会引起高阶模态振动，同时，由于转子陀螺效应的影响，振动频率会随转速发生变化，给控制器设计带来不确定性. 针对这个现象，本文设计考虑频率加性不确定性的H∞ 鲁棒控制器，在实验中实现了全转速范围内对高阶模态振动的抑制：
1） 转子上螺栓连接形成的界面接触可以看作无质量均布弹簧单元连接，转子在悬浮过程中，在接触界面之间形成多自由度的微幅振动，在弹簧单元的作用下放大为系统的内部扰动，以正反馈的形式作用于闭环控制中，激发系统的模态振动. 同时，陀螺效应会导致转子振动频率随转速变化.
2） 针对界面接触激发的模态振动频率随转速变化的现象，在被控对象构建的过程中通过加性不确定性将振动频率不确定性引入，从而设计H∞ 鲁棒控制器，H∞ 控制器在低频段具有明显的积分效应，在中频段可以实现类似于PD控制器的频响特性，在四阶弯曲模态频率范围内，产生类似于宽频带阻滤波器的频响特性来抑制磁悬浮轴承转子系统的弯曲模态振动.
-
图 6 磁悬浮轴承转子系统静态悬浮仿真响应
Figure 6. Static levitation simulation response of magnetic bearing-rotor system
图 7 磁悬浮轴承转子系统静态悬浮实验响应
Figure 7. Static levitation experiment response of magnetic bearing-rotor system
图 13 被控对象扫频试验原理框图
Figure 13. Sweep frequency experiment principle for controlled object
表 1 径向磁悬浮轴承结构参数
Table 1. Structural parameters of radial magnetic bearing
参数 取值 单个磁极匝数 N/匝 75 单个磁极面积 A/m2 4.05 × 10－4 偏置电流 I0/A 2 单边气隙 C0/mm 0.25 
下载: 导出CSV
表 2 理论和试验弯曲模态频率
Table 2. Theoretical and experimental bending mode frequencies
Hz 弯曲模态阶数 理论值 试验值 1 91.26 91.83 2 255.14 257.12 3 526.41 519.53 4 816.02 817.46 5 1046.77 1032.57
下载: 导出CSV
-
[1] SCHWEITZER G, MASLEN E, BLEULER H, et al. Magnetic bearing:theory, design and application to rotating machinery[M]. Berlin:Springer-Verilog, 2009:11-12. [2] YANNICK P,JEFFREY S,王小明. 应用API617第8版配备磁悬浮轴承的透平膨胀机-压缩机[J]. 风机技术,2016,58(5): 6-18. [3] HUTTERER M, SCHRÖDL M. Modeling and mu-synthesis control of a flexible rotor stabilized by active magnetic bearings including current free control[J]. Journal of Sound and Vibration, 2023, 546:117439.1-117439.18. [4] INOUE T, LIU J, ISHIDA Y, et al. Vibration control and unbalance estimation of a nonlinear rotor system using disturbance observer[J]. Journal of Vibration and Acoustics, 2009, 131(3): 031010.1-031010.8. [5] LI K X, PENG C, DENG Z Q, et al. Field dynamic balancing for active magnetic bearings supporting rigid rotor shaft based on extended state observer[J]. Mechanical Systems and Signal Processing, 2021, 158: 107801.1-107801.14. [6] TANG E Q, HAN B C, ZHANG Y. Optimum compensator design for the flexible rotor in magnetically suspended motor to pass the first bending critical speed[J]. IEEE Transactions on Industrial Electronics, 2016, 63(1): 343-354. doi: 10.1109/TIE.2015.2472534 [7] JIN C W, XIONG F, BAO Z P, et al. Research on modal vibration suppression of maglev steel strip based on phase compensator[J]. Journal of Sound and Vibration, 2018, 434: 78-91. doi: 10.1016/j.jsv.2018.07.043 [8] PENG C, ZHENG S Q, HUANG Z Y, et al. Complete synchronous vibration suppression for a variable-speed magnetically suspended flywheel using phase lead compensation[J]. IEEE Transactions on Industrial Electronics, 2018, 65(7): 5837-5846. doi: 10.1109/TIE.2017.2782204 [9] ZHENG S Q, HAN B C, WANG Y G, et al. Optimization of damping compensation for a flexible rotor system with active magnetic bearing considering gyroscopic effect[J]. IEEE/ASME Transactions on Mechatronics, 2015, 20(3): 1130-1137. doi: 10.1109/TMECH.2014.2344664 [10] ROY H K, DAS A S, DUTT J K. An efficient rotor suspension with active magnetic bearings having viscoelastic control law[J]. Mechanism and Machine Theory, 2016, 98: 48-63. doi: 10.1016/j.mechmachtheory.2015.11.012 [11] TANG E Q, FANG J C, HAN B C. Active vibration control of the flexible rotor in high energy density magnetically suspended motor with mode separation method[J]. Journal of Engineering for Gas Turbines and Power, 2015, 137(8): 082503.1-082503.10. [12] HAN X M, ZHOU J, ZHOU Y. Analysis and suppression of self-excited vibration of flexible rotor AMBs system[J]. Journal of Vibration Engineering & Technologies, 2021, 9(8): 1911-1922. [13] 魏彤,房建成. 基于双频Bode图设计磁悬浮弹性转子陷波器[J]. 光学精密工程,2008,16(5): 789-796.WEI Tong, FANG Jiancheng. Design of magnetically suspended elastic rotor Notch filter based on two-frequency Bode diagram[J]. Optics and Precision Engineering, 2008, 16(5): 789-796. [14] 崔培玲,赵光再,房建成,等. 基于相移陷波器的磁轴承不平衡振动全频自适应控制[J]. 振动与冲击,2015,34(20): 16-20,36.CUI Peiling, ZHAO Guangzai, FANG Jiancheng, et al. Adaptive control of unbalance vibration for magnetic bearings based on phase-shift Notch filter within the whole frequency range[J]. Journal of Vibration and Shock, 2015, 34(20): 16-20,36. [15] MUSHI S E, LIN Z, ALLAIRE P E. Stability analysis for a flexible rotor on active magnetic bearings subject to aerodynamic loads[C]//Proceedings of the 12th international symposium on magnetic bearings. Yokohama:[s.n.], 2010:22-25. [16] RAN S L, HU Y F, WU H C. Design, modeling, and robust control of the flexible rotor to pass the first bending critical speed with active magnetic bearing[J]. Advances in Mechanical Engineering, 2018, 10(2): 1-13. [17] 谭大力,陈进,廖明夫,等. 圆柱面配合激起的转子失稳振动研究[J]. 机械科学与技术,2014,33(12): 1786-1790.TAN Dali, CHEN Jin, LIAO Mingfu, et al. Instability caused by cylindrical surface fit in rotor system[J]. Mechanical Science and Technology for Aerospace Engineering, 2014, 33(12): 1786-1790. [18] 曾瑶,陈亮,蒋云帆,等. 圆柱面配合对柔性转子稳定性的影响[J]. 燃气涡轮试验与研究,2019,32(2): 42-48. doi: 10.3969/j.issn.1672-2620.2019.02.008ZENG Yao, CHEN Liang, JIANG Yunfan, et al. Analysis on the impact of cylindrical surface fit on flexible rotor[J]. Gas Turbine Experiment and Research, 2019, 32(2): 42-48. doi: 10.3969/j.issn.1672-2620.2019.02.008 [19] LIU Y, LIU H, YI J, et al. Investigation on the stability and bifurcation of a rod-fastening rotor bearing system[J]. Journal of Vibration and Control, 2015, 21(14): 2866-2880. doi: 10.1177/1077546313518817 [20] WU X L, JIAO Y H, CHEN Z B, et al. Establishment of a contact stiffness matrix and its effect on the dynamic behavior of rod-fastening rotor bearing system[J]. Archive of Applied Mechanics, 2021, 91(7): 3247-3271. doi: 10.1007/s00419-021-01963-9 [21] 魏彤,房建成. 磁悬浮控制力矩陀螺高速转子高频自激振动的抑制[J]. 宇航学报,2006,27(2): 291-296. doi: 10.3321/j.issn:1000-1328.2006.02.029WEI Tong, FANG Jiancheng. Self-excited vibration depression of high-speed rotor in magnetically suspended control moment gyroscope[J]. Journal of Astronautics, 2006, 27(2): 291-296. doi: 10.3321/j.issn:1000-1328.2006.02.029 [22] ZHOU Y, ZHOU J, MAHFOUD J, et al. Modelling and Validation of Rotor-Active Magnetic Bearing System Considering Interface Contact[C]//Proceedings of the 11th IFToMM International Conference on Rotordynamics. Mechanisms and Machine Science. Berlin:Springer, 2023:374-390. 期刊类型引用(0)
其他类型引用(1)
-
下载:
下载:
点击查看大图
计量
- 文章访问数: 323
- HTML全文浏览量: 212
- PDF下载量: 92
- 被引次数: 1