Comparison of Shear Behaviors of Different Concrete-Filled High-Strength Steel Tubes
-
摘要:
为研究剪跨比和混凝土强度对高强方钢管混凝土试件抗剪性能的影响,共设计16个高强方钢管混凝土试件. 通过试验获得试件的破坏模式和剪力-位移曲线,对比剪跨比、核心混凝土强度等参数对破坏模式、剪力-位移曲线、剪力-剪应变曲线、抗剪强度和刚度的影响. 研究结果表明:与普通钢管混凝土试件一致,剪跨比是控制高强方钢管混凝土试件破坏模式的主要参数,当剪跨比为0.2或0.5时,发生剪切破坏;当剪跨比为0.8或1.0时,发生弯剪破坏;采用超高性能混凝土填充高强方钢管(剪跨比为1.0),试件的变形能力降低61.4%,抗剪强度和刚度分别提高了38.9%和85.7%,且有效延缓钢管局部屈曲和降低试件的破坏程度;核心混凝土主斜裂缝产生的倾角随剪跨比增大而减小,但不受混凝土强度影响. 本文抗剪强度试验值/抗剪强度计算值(
V exp/V u)的均值为0.97,方差为0.03,精度高,离散性小,认为该公式可较准确地预测高强钢管混凝土的抗剪强度.Abstract:A total of 16 concrete-filled high-strength steel tube specimens were designed to evaluate the effects of shear-to-span ratio and concrete strength on the shear behavior of concrete-filled high-strength steel tube specimens. The failure mode and shear-displacement curve of the specimens were obtained through the test, and the effects of shear-to-span ratio, core concrete strength, and other parameters on the failure mode, shear-displacement curve, shear-shear strain curve, shear strength, and shear stiffness were compared. The results show that similar to conventional concrete-filled steel tube specimens, the shear-to-span ratio is the key parameter controlling the failure mode of the concrete-filled high-strength steel tube specimens. When the shear-to-span ratio is 0.2 or 0.5, shear failure occurs; when the shear-to-span ratio is 0.8 or 1.0, shear-flexural failure occurs. By implementing ultra-high performance concrete (UHPC), the deformation capacity of the concrete-filled high-strength steel tube specimens (with a shear-to-span ratio of 1.0) is reduced by 61.4%. However, shear strength and stiffness increase by 38.9% and 85.7%, respectively. Additionally, local buckling of the steel tubes is effectively delayed, and the damage degree of the specimens is reduced. The inclination angle of the main diagonal cracks in the core concrete decreases with an increase in the shear-to-span ratio but is not affected by the concrete strength. The average value of tested shear strength/calculated shear strength (
V exp/V u) is 0.97, with a deviation of 0.03, showing higher accuracy and smaller dispersion. It is considered that the formula can accurately predict the shear strength of concrete-filled high-strength steel tubes.-
Key words:
- concrete-filled steel tube /
- high-strength steel tube /
- shear behavior /
- failure mode /
- design method
-
钢管混凝土构件是指将混凝土填充至钢管而形成的一种组合构件[1][2]. 随着材料科学的发展和技术的进步,钢材和混凝土的强度得到了极大的提高[3]. 例如,钢材的屈服应力可超过960 MPa[4],混凝土的抗压强度可高达800 MPa[5]. 将高强材料应用于组合结构,既可有效减少结构的截面尺寸,又可减轻结构的自重[6-7]. 目前,高强钢管混凝土的应用已有不少,如:Abeno Harukas大楼(日本大阪)和Latitude大楼(澳大利亚悉尼)均采用了高强钢管混凝土柱.
国内外学者对普通钢管混凝土构件的抗剪性能进行了广泛而深入的研究:黄勇等[8]分析不同截面类型的钢管混凝土在单调加载条件下的抗剪性能,发现截面类型对钢管混凝土的相对变形有一定影响;李志强等[9]对10个矩形钢管混凝土进行弯-剪性能试验研究,并基于斜压杆机制提出矩形钢管混凝土受剪承载力计算公式;史艳莉等[10]对8个无端板矩形钢管混凝土进行剪切性能试验研究,揭示截面尺寸和剪跨比对抗剪承载力的影响规律;丁威[11]以剪跨比、长宽比、加载角度为主要参数研究矩形钢管混凝土的抗剪性能,并提出抗剪承载力计算公式;Mansouri[12]研究约束系数、剪跨比和轴压比对钢管混凝土抗剪性能的影响,提出圆钢管混凝土抗剪强度计算方法;Lehman等[13-14]结合试验和有限元分析,确定钢管混凝土的破坏模式(弯曲、剪切或黏结),开发了一个简单的数值表达式来区分弯曲和剪切破坏.
然而,目前对高强方钢管混凝土的研究主要集中于轴压、偏压和受弯方面[15-16]. Yan等[17]对高强方钢管超高性能混凝土柱的轴压性能进行研究,发现该组合柱表现出良好的延性特性;Hu等[18]结合试验研究和数值模拟,探索矩形高强钢管超高性能混凝土柱在偏心荷载下的性能,认为在超高性能混凝土中掺入钢纤维可以提高组合柱的承载力和延性;Lai等[19]采用试验研究和数值模拟相结合的方法,研究矩形高强钢管超高性能混凝土梁的受弯性能,获得典型的破坏模式,并确定了关键参数.
上述研究成果对深入了解钢管混凝土组合构件在不同荷载条件下的力学性能具有重要学术价值. 然而,仍需要进一步开展系统性的研究,以探索高强方钢管混凝土在剪切作用下的性能. 基于此,本文设计16个高强方钢管混凝土试件,考察剪跨比和混凝土强度对抗剪性能的影响,对比不同强度混凝土填充高强方钢管的优势与弊端. 最后,对我国钢管混凝土结构技术规程[20]、美国路桥设计规范[21]、欧洲规范[22]和华盛顿桥梁设计规范[23]等提出的计算公式进行适用性评估,为高强方钢管混凝土的应用提供试验数据与理论支持.
1. 试验概况
1.1 试件设计
本文设计了16个高强方钢管混凝土试件进行抗剪性能试验研究,其中,包括8个高强方钢管超高性能混凝土试件、4个高强方钢管高强混凝土试件和4个高强方钢管普通混凝土试件. 试验主要研究参数:剪跨比(a/H,a为试件的剪跨长,H为试件界面高度)和混凝土强度(fc1). 试件主要参数如表1所示. 表1中:试件编号部分HS代表高强方钢管,CC代表普通强度混凝土,HC代表高强混凝土,UC代表超高性能混凝土,数字0%、2%表示钢纤维体积掺量,数字0.2、0.5、0.8、1.0表示剪跨比;L为钢管长度,B为钢管截面宽度,fy为钢材屈服应力,t为钢管壁厚,ρ为钢纤维体积掺量,Es和Ec分别为钢材和混凝土的弹性模量.
表 1 试件主要参数Table 1. Main parameters of specimens序号 试件编号 L/mm B/mm H/mm ρ/% fy/MPa fc1/MPa t/mm Es/GPa Ec/GPa 1 HSCC-0.2 1000 120 120 747 30 3 206 32.7 2 HSCC-0.5 1000 120 120 747 30 3 206 32.7 3 HSCC-0.8 1000 120 120 747 30 3 206 32.7 4 HSCC-1.0 1000 120 120 747 30 3 206 32.7 5 HSHC-0.2 1000 120 120 782 90 3 206 43.4 6 HSHC-0.5 1000 120 120 782 90 3 206 43.4 7 HSHC-0.8 1000 120 120 782 90 3 206 43.4 8 HSHC-1.0 1000 120 120 782 90 3 206 43.4 9 HSUC-0%-0.2 1000 120 120 747 115 3 206 41.9 10 HSUC-0%-0.5 1000 120 120 747 115 3 206 41.9 11 HSUC-0%-0.8 1000 120 120 747 115 3 206 41.9 12 HSUC-0%-1.0 1000 120 120 747 115 3 206 41.9 13 HSUC-2%-0.2 1000 120 120 2 775 135.4 3 206 44.8 14 HSUC-2%-0.5 1000 120 120 2 775 135.4 3 206 44.8 15 HSUC-2%-0.8 1000 120 120 2 775 135.4 3 206 44.8 16 HSUC-2%-1.0 1000 120 120 2 775 135.4 3 206 44.8 本文包含4种强度混凝土:钢纤维体积掺量为2%的超高性能混凝土(135.4 MPa)、不掺入钢纤维的超高性能混凝土(115 MPa)、高强混凝土(90 MPa)和普通混凝土(30 MPa). 所有强度混凝土的配合比见表2. 本文试验选用P.O 42.5普通硅酸盐水泥;硅灰粒径为0.1~0.2 μm,SiO2含量高达90%,密度为
2000 kg/m3,比表面积为19 × 10−1 m2/kg;CX-8型高效减水剂,减水率在25%以上;石英砂颗粒级配有4种,从小到大分别为400、40~70、20~40、10~20;直线型钢纤维直径和长度分别为0.2 mm和13.0 mm,抗拉强度高达2000 MPa.表 2 混凝土配合比Table 2. Concrete mix proportionskg/m3 混凝土类别 水 水泥 硅灰 粗骨料 细骨料 减水剂 钢纤维体积掺量/% 普通(C30) 180.0 370.0 1042.0 766.0 7.40 高强(C90) 156.8 579.4 102.2 1050.8 617.2 15.48 UHPC (无钢纤维) 221.7 852.5 255.7 920.7 80.1 21.30 UHPC (2% 钢纤维) 221.7 852.5 255.7 920.7 80.1 21.30 2 钢材与混凝土的力学性能见表1. 需要说明的是,本文所用高强钢是指屈服应力大于或等于525 MPa的钢材,高强混凝土和超高性能混凝土分别是指抗压强度大于或等于70 MPa和100 MPa的混凝土[24].
1.2 试验装置及加载制度
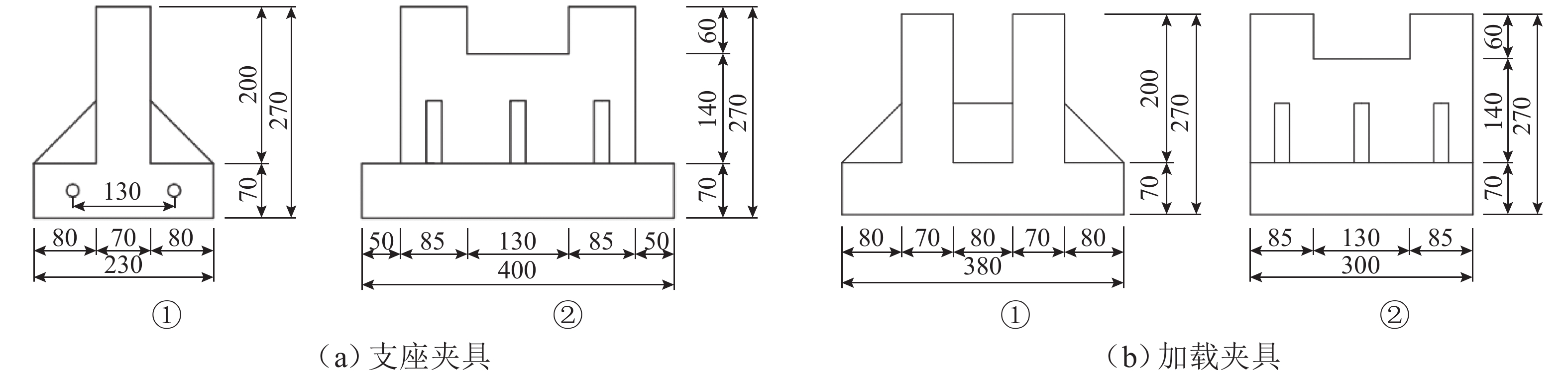
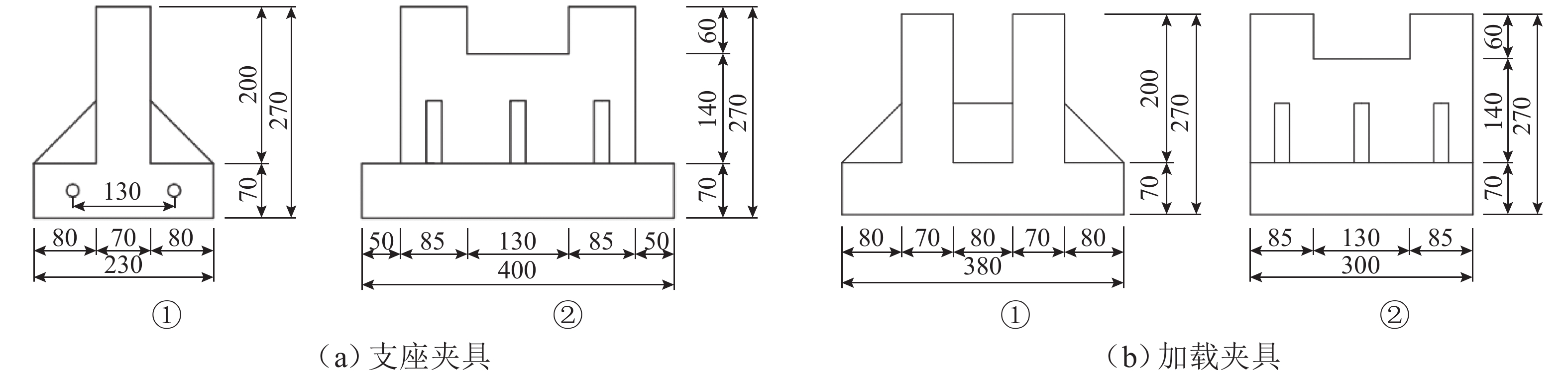
本试验压力机量程为
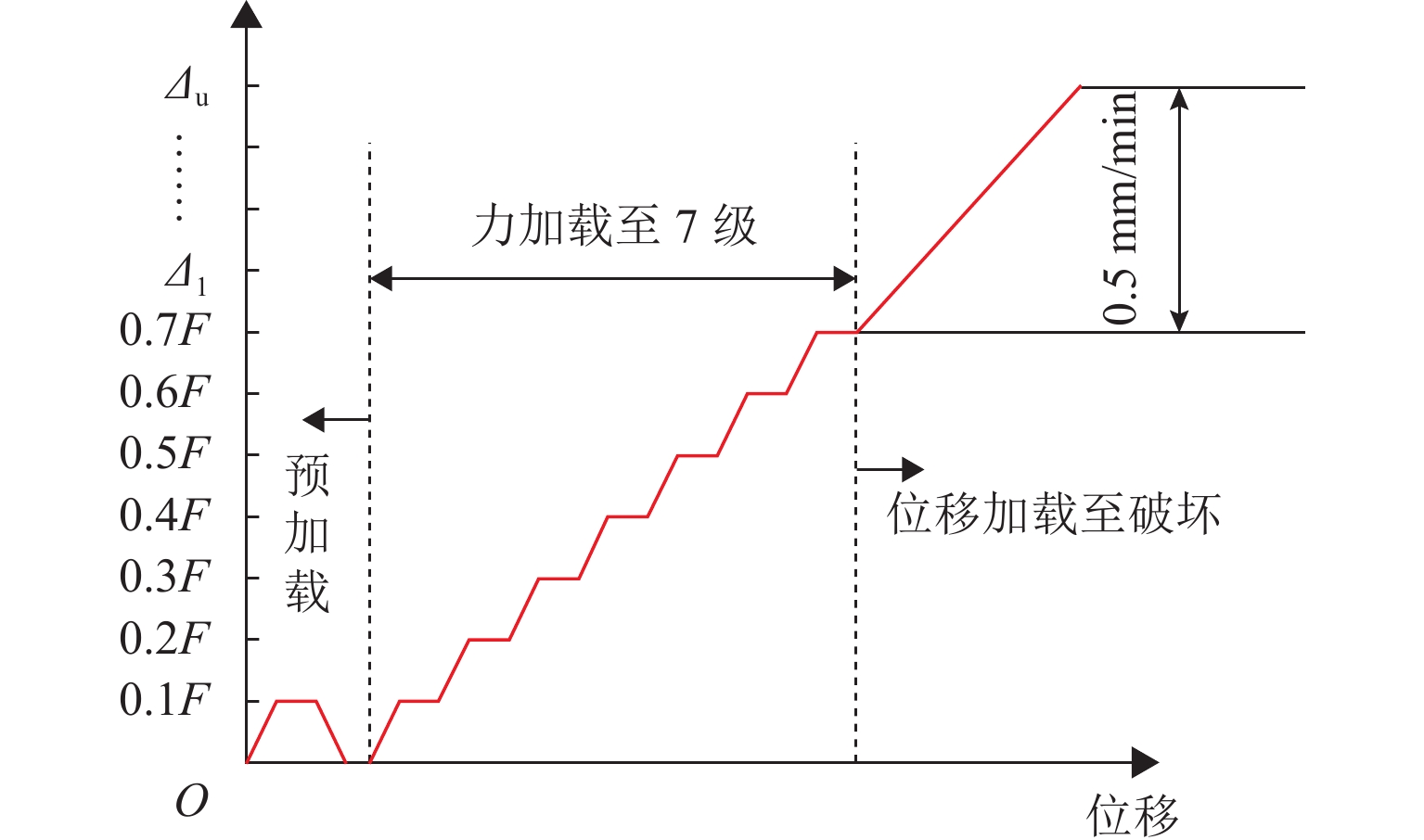
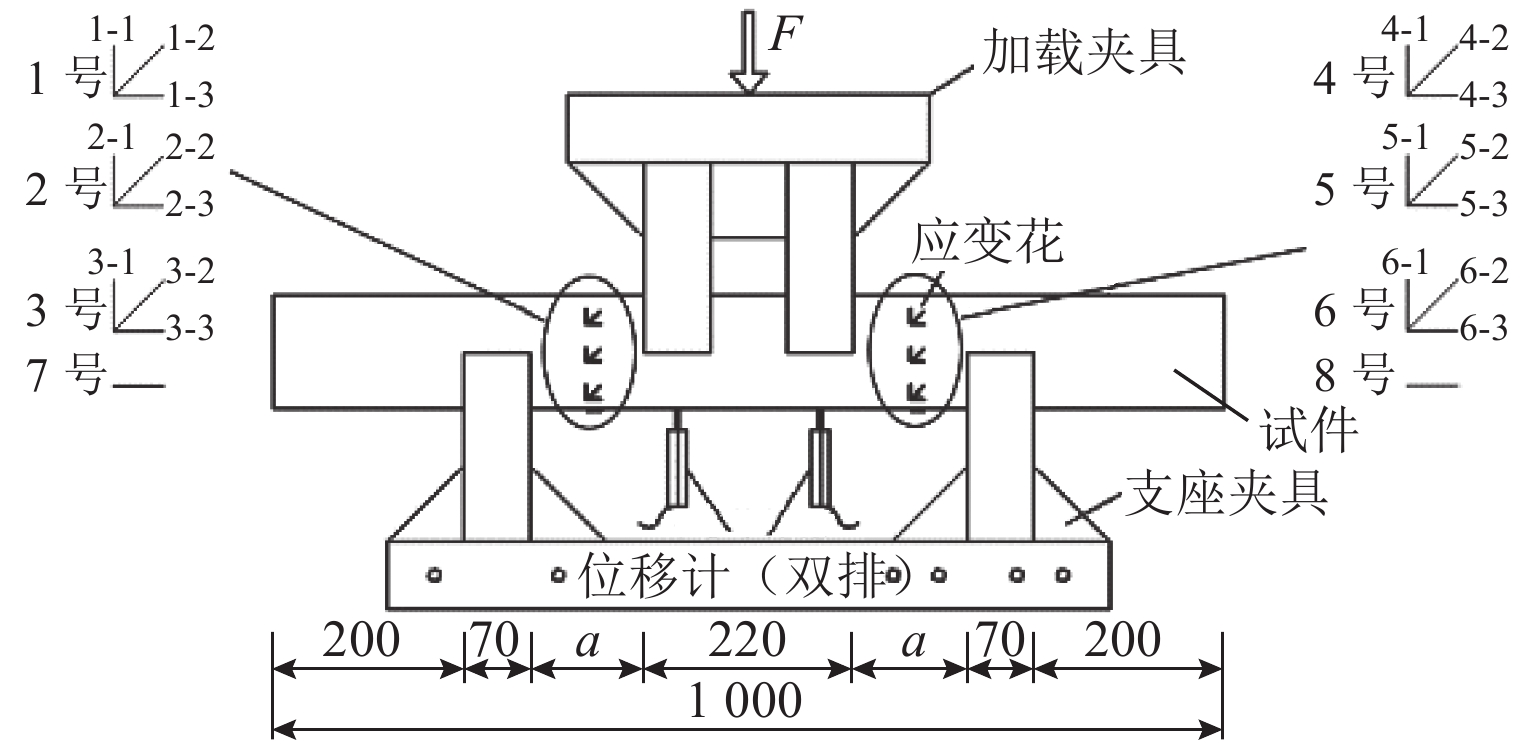
5000 kN,采用荷载传感器采集荷载数据,利用JM-3813静态应变测试分析系统采集位移计和电阻应变片的数据,试验加载装置如图1所示. 为避免试验时支座夹具产生滑移以及方便调节剪跨(即调节2个支座之间的相对距离),在2个支座夹具均留有螺栓孔,用钢条将其连接,加载夹具和支座夹具详见图2. 加载夹具与支座夹具的刚度均满足试验要求.试验前,先进行预加载(施加的荷载约为0.1F,F为预估峰值荷载),以检验数据采集系统和减少试验误差. 试验采用分级加载制度,即由力和位移加载两部分组成,如图3所示. 图中,Δ1和Δu分别为初始位移和极限位移. 在弹性阶段,力控制加载,每级加载约为0.1F,加载速度为0.5 kN/s. 当加载到0.7F后,转为位移控制加载,速度为0.5 mm/min. 出现以下3种情况之一,停止加载:1) 试件产生明显的剪切变形,无法继续加载;2) 钢管局部鼓曲变形过大导致数据异常;3) 试件的承载力下降到0.85F.
测点布置如图4所示,其中包括4个位移计、6个应变花和2个应变片,共计24个测点. 位移计用来测量试件的剪切位移,布置在加载点所对应构件的正下方,前后各2个呈双排对称布置,试验结果取4个位移计的算术平均值. 左右两个剪跨区分别对称布置3个应变花(左、右两侧编号分别为1、2、3和4、5、6)和1个纵向应变片,总计20个应变数据. 钢管底部剪跨区的纵向应变片编号为7和8. 以1号应变花为例说明应变花3个方向的应变片编号:0°、45° 和90° 方向的应变片编号分别为1-3、1-2、1-1.
2. 试验结果及分析
所有试件试验结果如表3所示. 表中:Vexp为抗剪强度试验值,Δy为屈服位移,Δu为极限位移,K为抗剪刚度. Δu取试件85%下降荷载所对应的位移,若荷载未出现显著下降,其值取破坏时所对应的位移.
表 3 试验结果Table 3. Test results试件编号 Vexp/
kNΔy/
mmΔu/
mmK/
(kN·mm−1)破坏模式 HSCC-0.2 536 0.9 9.5 573 剪切破坏 HSCC-0.5 458 2.3 28.4 201 剪切破坏 HSCC-0.8 409 2.4 29.7 168 弯剪破坏 HSCC-1.0 396 4.4 >30.0 91 弯剪破坏 HSHC-0.2 635 1.1 7.2 598 剪切破坏 HSHC-0.5 536 2.1 22.6 258 剪切破坏 HSHC-0.8 466 2.5 27.7 181 弯剪破坏 HSHC-1.0 427 2.9 >30.0 148 弯剪破坏 HSUC-0%-0.2 741 1.1 14.5 706 剪切破坏 HSUC-0%-0.5 601 1.4 27.8 429 剪切破坏 HSUC-0%-0.8 533 2.1 21.2 249 弯剪破坏 HSUC-0%-1.0 499 3.5 23.5 142 弯剪破坏 HSUC-2%-0.2 810 1.1 6.1 737 剪切破坏 HSUC-2%-0.5 638 1.6 14.2 438 剪切破坏 HSUC-2%-0.8 589 2.3 22.4 251 弯剪破坏 HSUC-2%-1.0 550 3.3 23.3 169 弯剪破坏 2.1 试件破坏模式
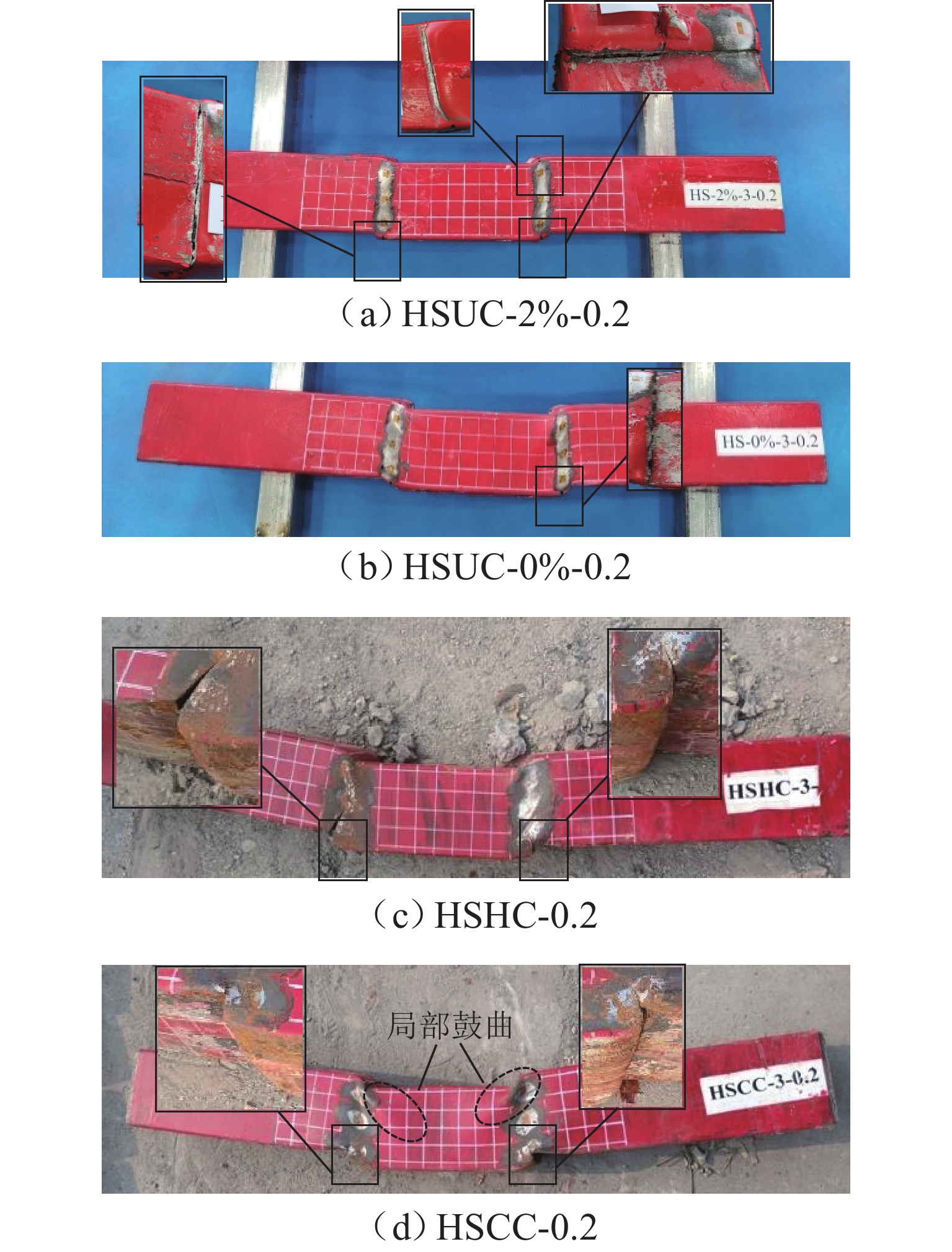
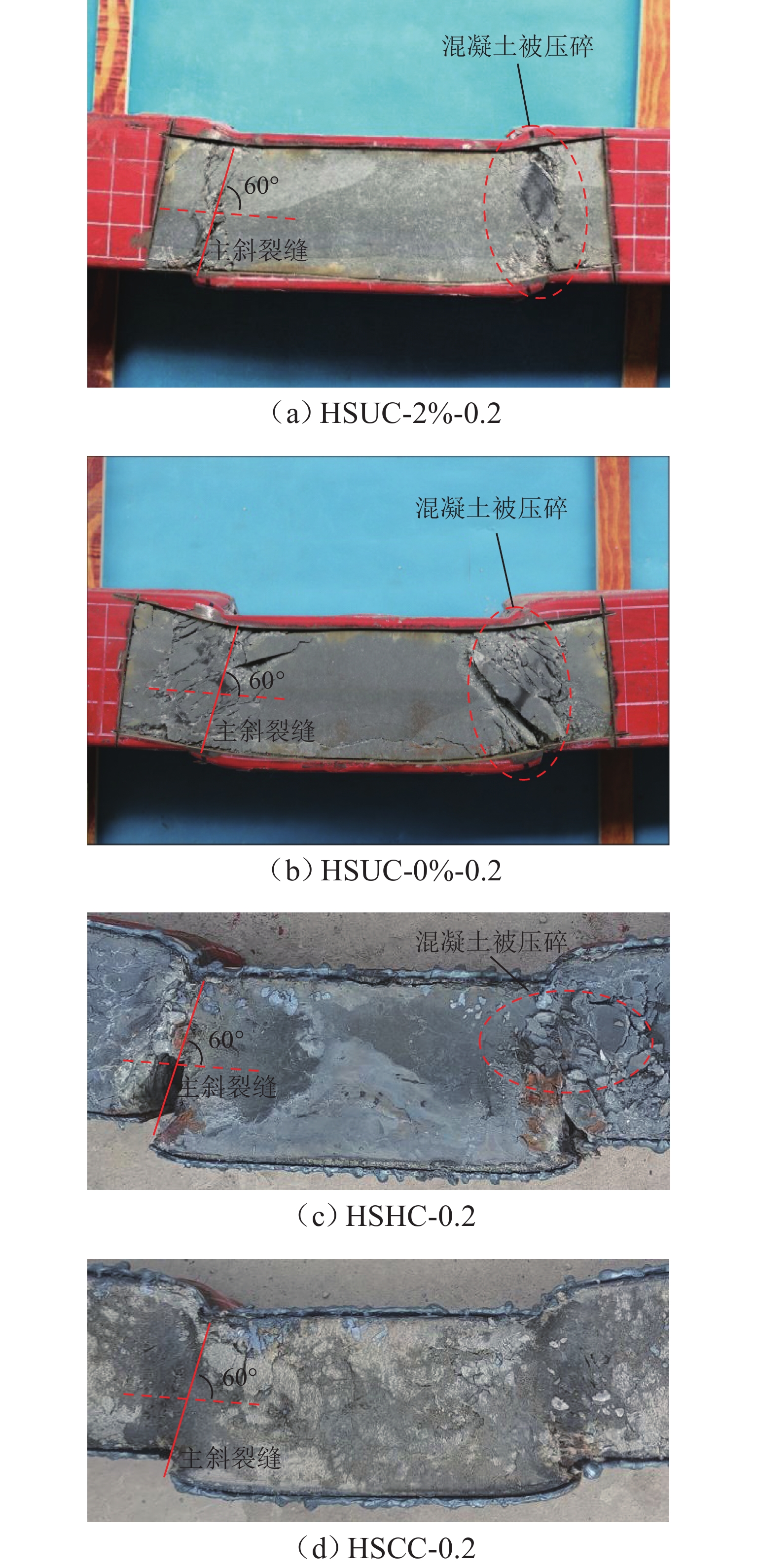
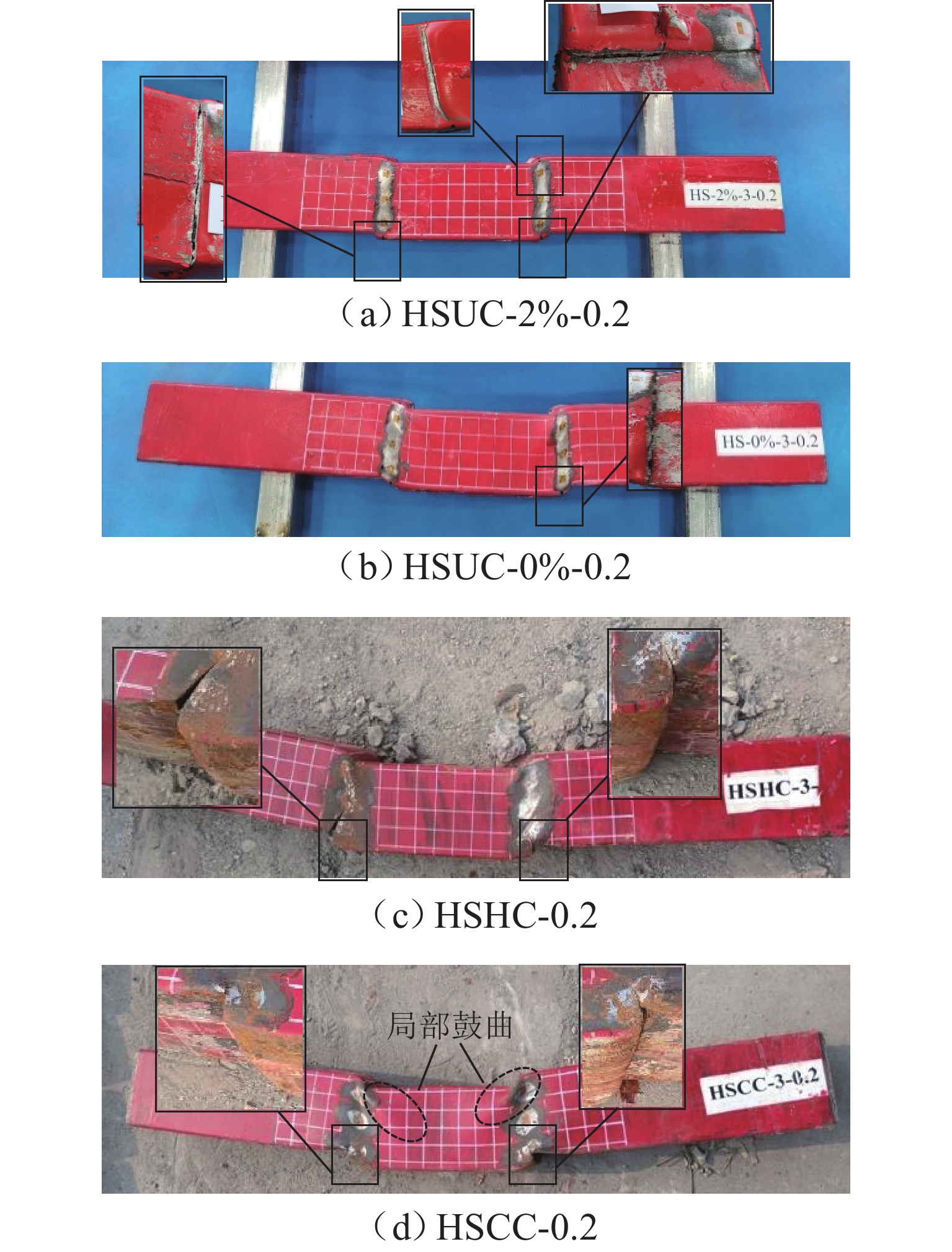
由表3可知,所有试件的破坏模式分为剪切破坏和弯剪破坏. 当剪跨比a/H=0.2或0.5时,试件发生剪切破坏;当剪跨比a/H=0.8或1.0时,试件发生弯剪破坏. 本节以典型破坏试件HSUC-2%-0.2、HSUC-0%-0.2、HSHC-0.2、HSCC-0.2、HSUC-2%-0.8、HSUC-0%-0.8、HSHC-0.8和HSCC-0.8为例,对比不同强度混凝土填充高强方钢管在剪切作用下的破坏模式.
HSUC-2%-0.2、HSUC-0%-0.2、HSHC-0.2、HSCC-0.2试件钢管的破坏模式如图5所示. 1) 这4组试件的共同点:① 发生剪切破坏,产生剪切错位变形;② 钢管均在支座处被撕裂. 2) 这4组试件的不同点:试件HSHC-0.2与HSCC-0.2钢管在支座处出现明显的鼓曲变形,而试件HSUC-2%-0.2和HSUC-0%-0.2的支座处鼓曲变形较小,可忽略不计,试件HSCC-0.2在加载点附近也出现了明显的鼓曲变形.
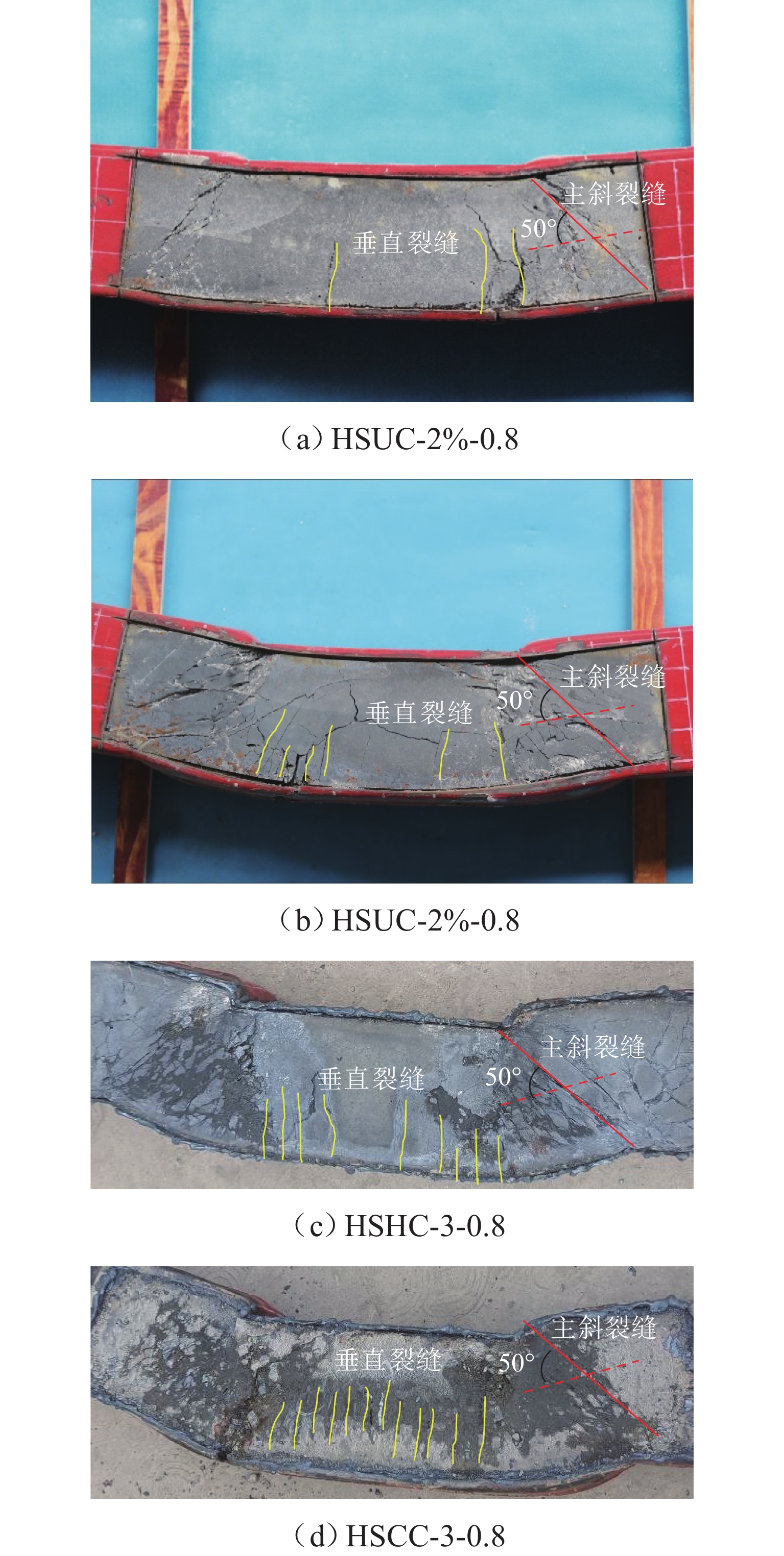
HSUC-2%-0.8、HSUC-0%-0.8、HSHC-0.8和HSCC-0.8试件钢管的破坏模式如图6所示. 这4组试件的共同点:1) 发生弯剪破坏,产生弯曲变形;2) 钢管均在离支座96 mm处发生撕裂且沿着截面高度方向发展,在支座处未发现钢管被撕裂的现象. 这4组试件的不同点:试件HSHC-0.8和HSCC-0.8均在加载点附近出现明显的局部鼓曲,而试件HSUC-2%-0.8和HSUC-0%-0.8的加载点附近未出现明显的局部鼓曲.
通过对比不同强度混凝土填充高强方钢管在剪切作用下的破坏模式可以发现,剪跨比是控制高强方钢管混凝土试件破坏模式的主要参数,提高核心混凝土强度可以有效延缓钢管局部屈曲. 这是因为提高核心混凝土抗压强度,使得试件中性轴上移、钢管受压区应变减小,有效延缓外部钢管局部屈曲.
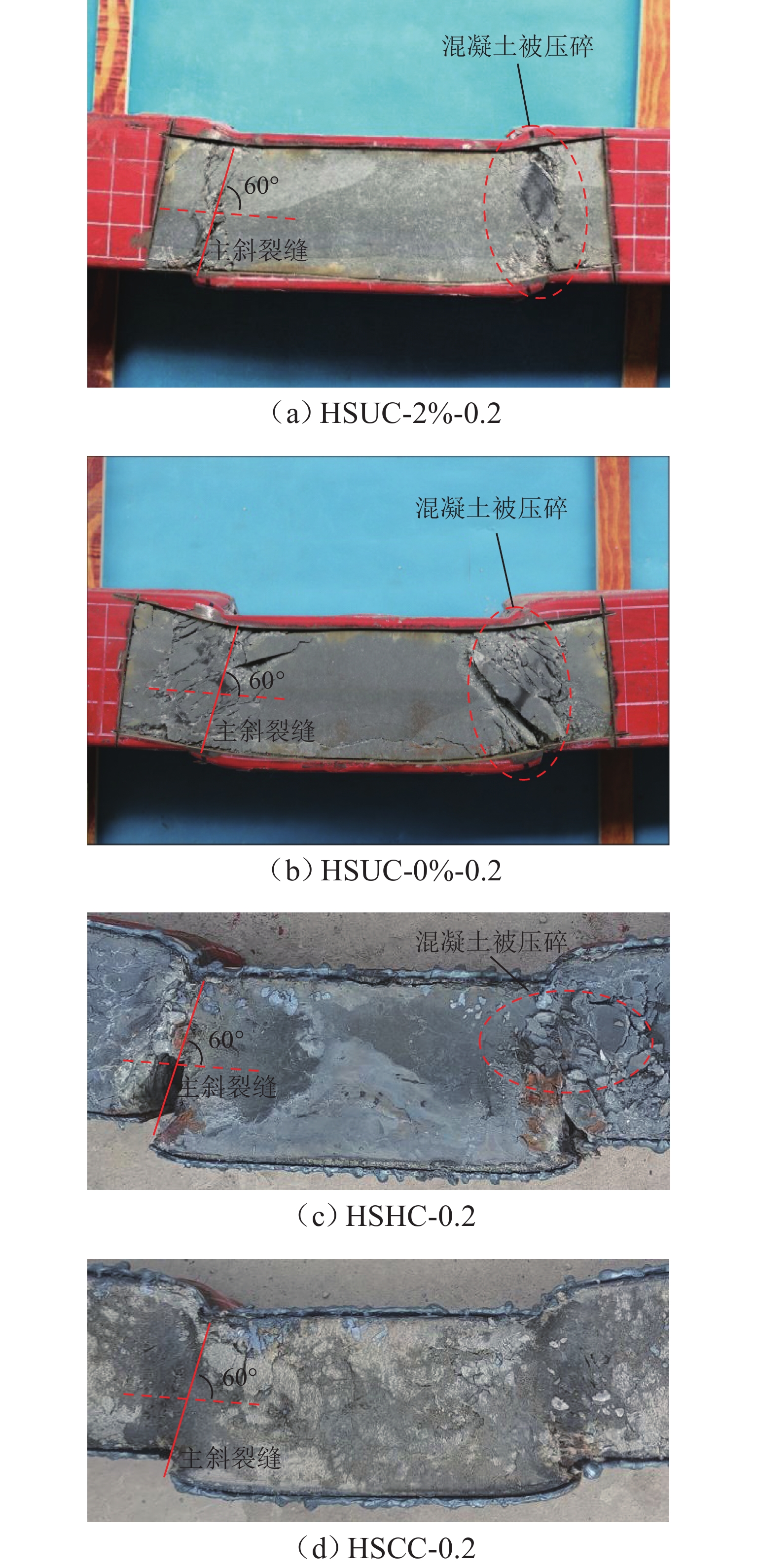
2.2 核心混凝土破坏模式
试验结束,对所有试件受力区段单侧外部钢管进行切割,观察并分析试件内部核心混凝土的破坏模式. 本节以典型破坏试件HSUC-2%-0.2、HSUC-0%-0.2、HSHC-0.2、HSCC-0.2、HSUC-2%-0.8、HSUC-0%-0.8、HSHC-0.8和HSCC-0.8为例,对比不同强度混凝土填充高强方钢管在剪切作用下其核心混凝土的破坏模式.
HSUC-2%-0.2、HSUC-0%-0.2、HSHC-0.2、HSCC-0.2试件核心混凝土的破坏模式如图7所示. 这4组试件的共同点:1) 剪跨区存在主斜裂缝;2) 核心混凝土主斜裂缝形成的倾角θ约为60°. 这4组试件的不同点:1) 高强混凝土和超高性能混凝土均沿着加载点与支座处的连线方向被压碎,但超高性能混凝土(钢纤维体积掺量为2%)的破坏程度最小;2) 超高性能混凝土(钢纤维体积掺量为2%)主斜裂缝的宽度远小于普通混凝土、高强混凝土和超高性能混凝土(未掺入钢纤维)主斜裂缝的宽度. 说明混凝土强度越高,其压溃程度与主斜裂缝宽度越小.
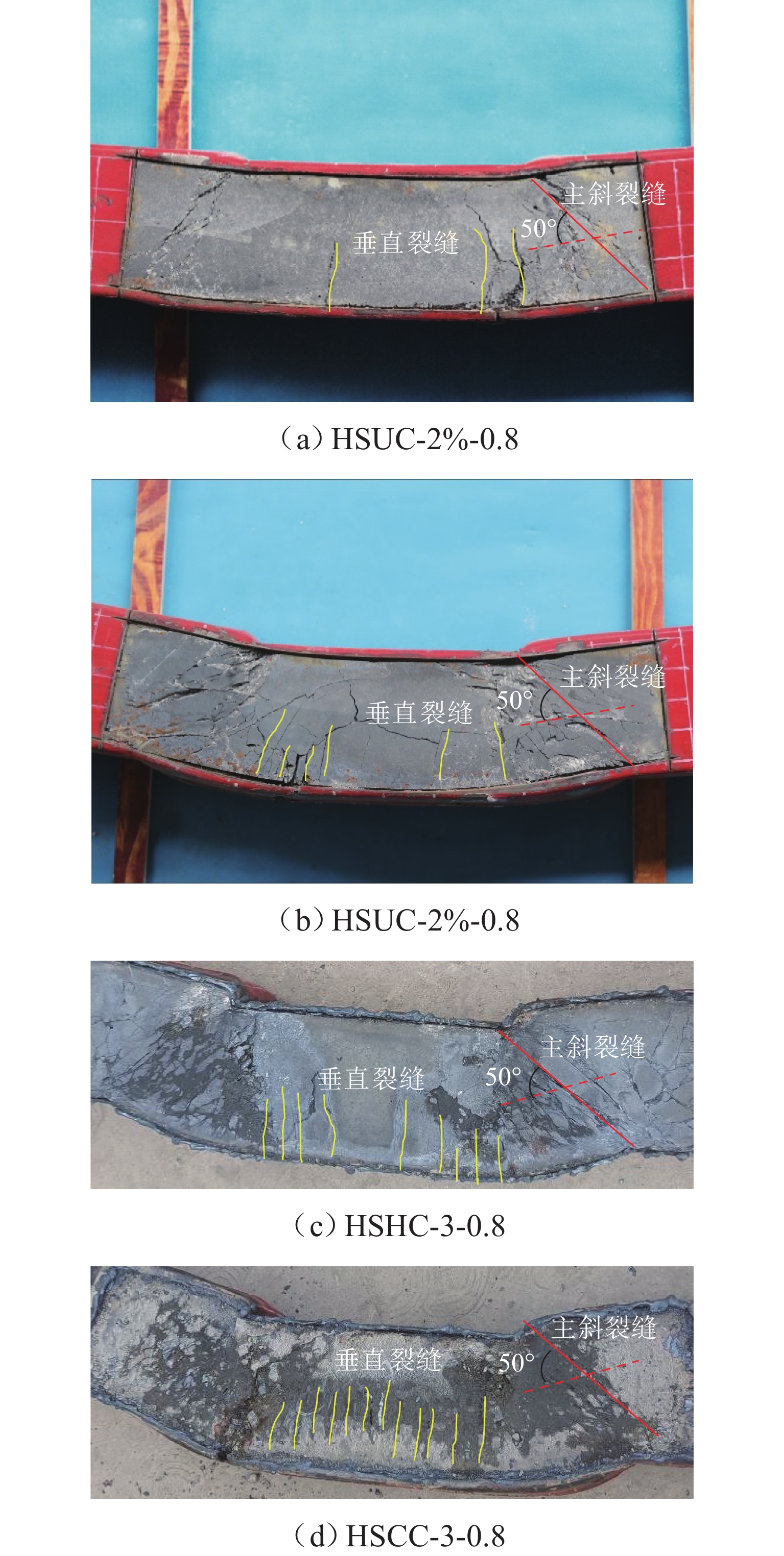
HSUC-2%-0.8、HSUC-0%-0.8、HSHC-0.8和HSCC-0.8试件核心混凝土的破坏模式如图8所示.
这4组试件的共同点:1) 剪跨区存在主斜裂缝;2) 核心混凝土的受拉区存在多条垂直裂缝;3) 核心混凝土主斜裂缝形成的倾角θ约为50°;4) 核心混凝土完整性较好. 这4组试件的不同点:1) 超高性能混凝土(钢纤维体积掺量为2%)主斜裂缝的宽度远小于普通混凝土、高强混凝土和超高性能混凝土(未掺入钢纤维)主斜裂缝的宽度;2) 超高性能混凝土(钢纤维体积掺量为2%)剪跨区的斜裂缝和受拉区的垂直裂缝远少于普通混凝土、高强混凝土和超高性能混凝土(未掺入钢纤维)剪跨区的斜裂缝以及受拉区的垂直裂缝.
通过对比不同强度混凝土填充高强方钢管在剪切作用下其核心混凝土的破坏模式可以发现,核心混凝土主斜裂缝形成的倾角θ随剪跨比增大而减小,混凝土强度对其无明显影响,但是提高混凝土强度可以降低其破坏程度.
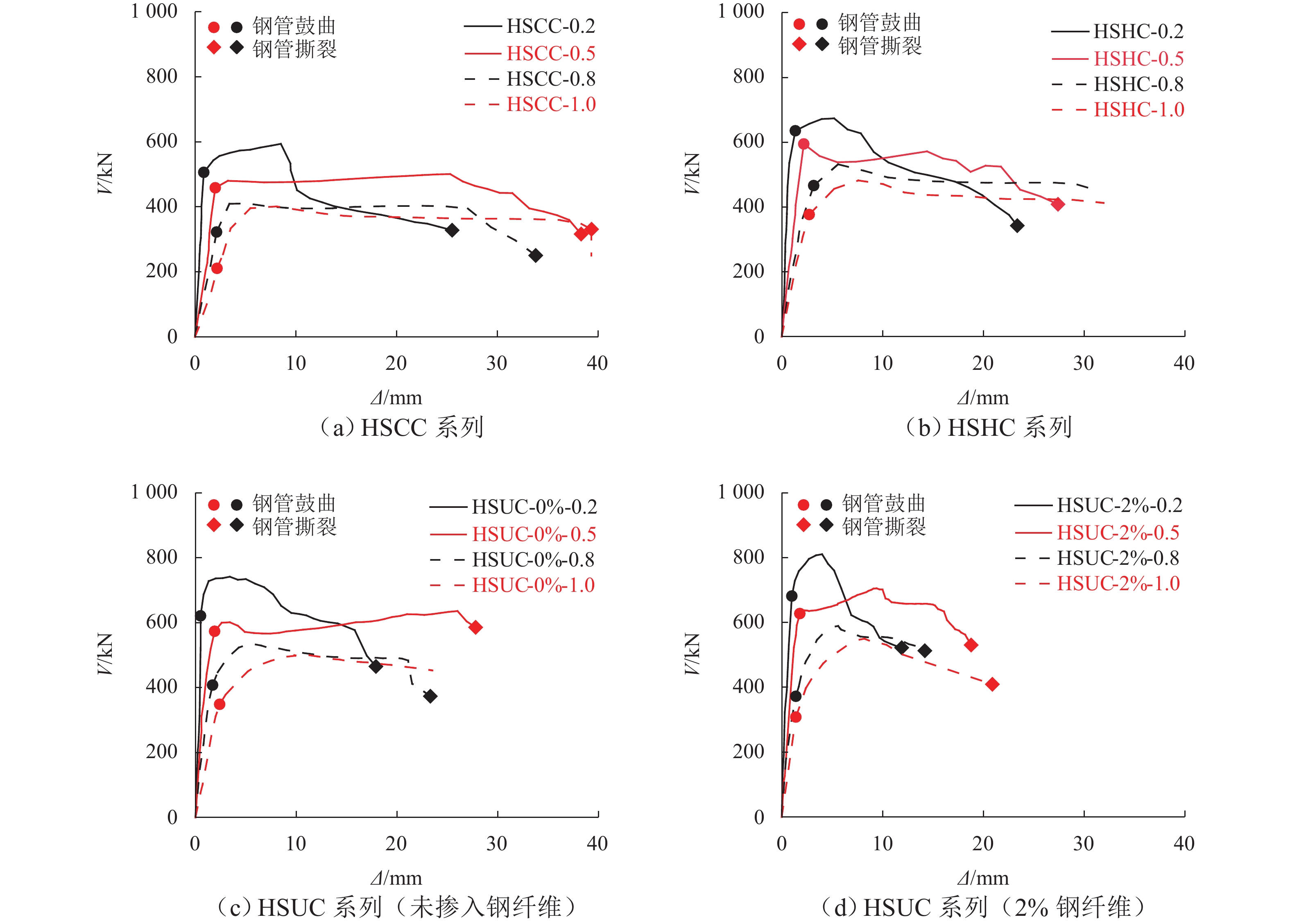
2.3 剪力-位移曲线
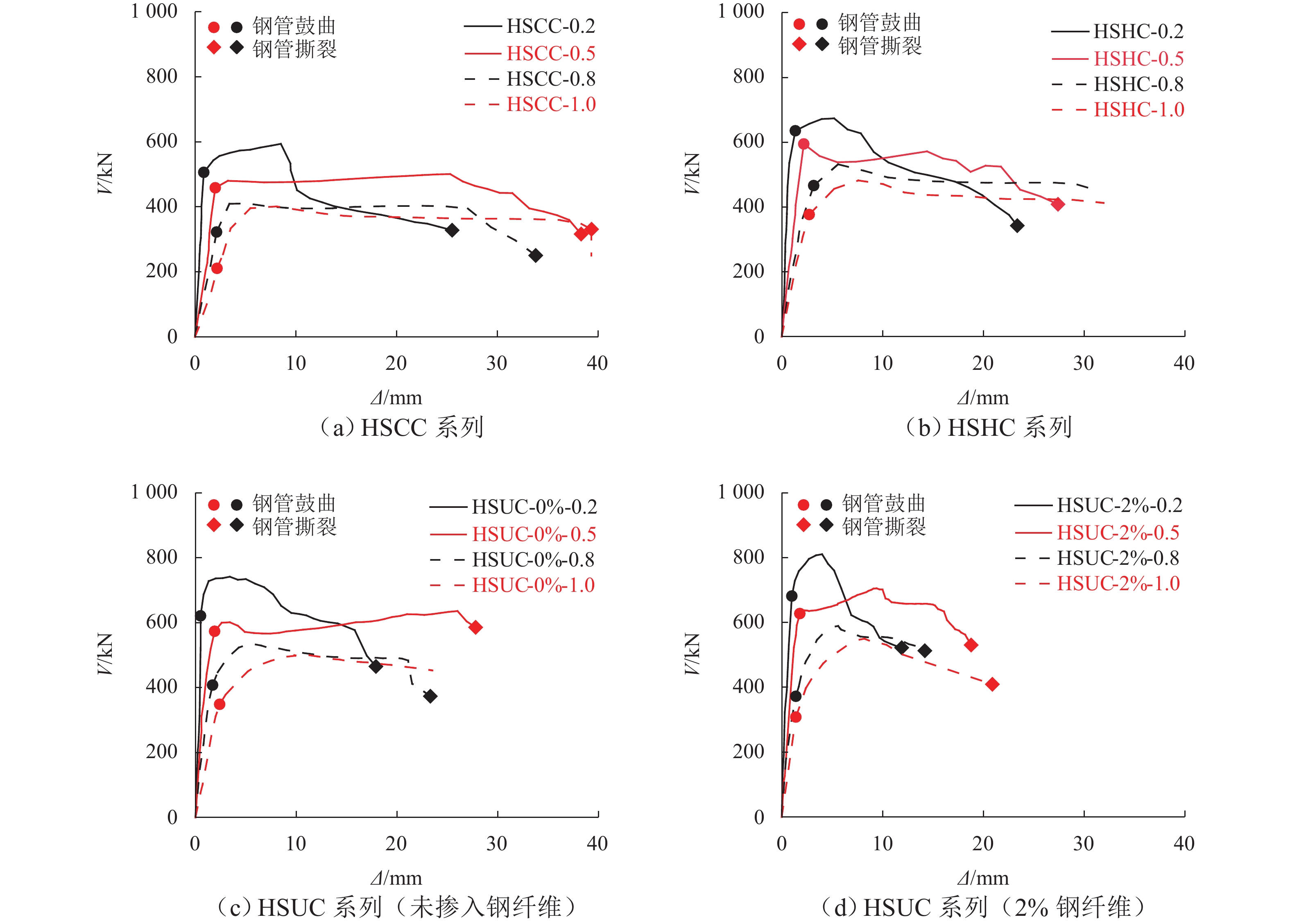
图9为所有试件剪力-位移关系曲线. 图中:Δ为位移,V为抗剪强度(剪力). 由图可知:所有曲线可大致分为弹性阶段和非弹性阶段,弹性阶段,在加载初期,曲线均呈线性增长;当剪跨比为0.2时,此阶段弹性刚度最大;当剪跨比由0.2增加至1.0时,弹性刚度逐渐减小,但提高混凝土强度,弹性刚度的降幅明显减小.
当钢管出现鼓曲,试件由弹性阶段逐渐转为非弹性阶段,核心混凝土强度较高的试件在达到极限抗剪强度后,出现明显的强度退化. 对于同系列试件,随着剪跨比增大,其变形能力显著增大,以HSCC系列为例,当剪跨比由0.2增长至1.0时,其极限位移增加了29.83 mm,非弹性阶段更长;对于不同系列的试件,HSCC系列试件的变形能力远高于其他3组试件的变形,例如,与试件HSCC-1.0的极限位移相比,试件HSHC-1.0、HSUC-0%-1.0和HSUC-2%-1.0的极限位移分别减小了7.39、15.83、24.13 mm. 说明剪跨比和混凝土强度是影响试件变形能力的主要因素,增大剪跨比和降低混凝土强度可以显著提高试件的变形能力. 这是因为剪跨比增大,试件的剪跨区段增大,变形能力提高. 另一方面,提高混凝土强度会降低其塑性,导致变形能力降低.
通过上述分析发现:剪跨比和混凝土强度是影响高强方钢管混凝土试件变形能力的主要参数;在混凝土强度相同的情况下,剪跨比较大的试件弹性刚度较小,非弹性工作阶段更长,变形能力更佳;在剪跨比相同的情况下,混凝土强度越高,弹性刚度越大,非弹性工作阶段越短,变形能力降低.
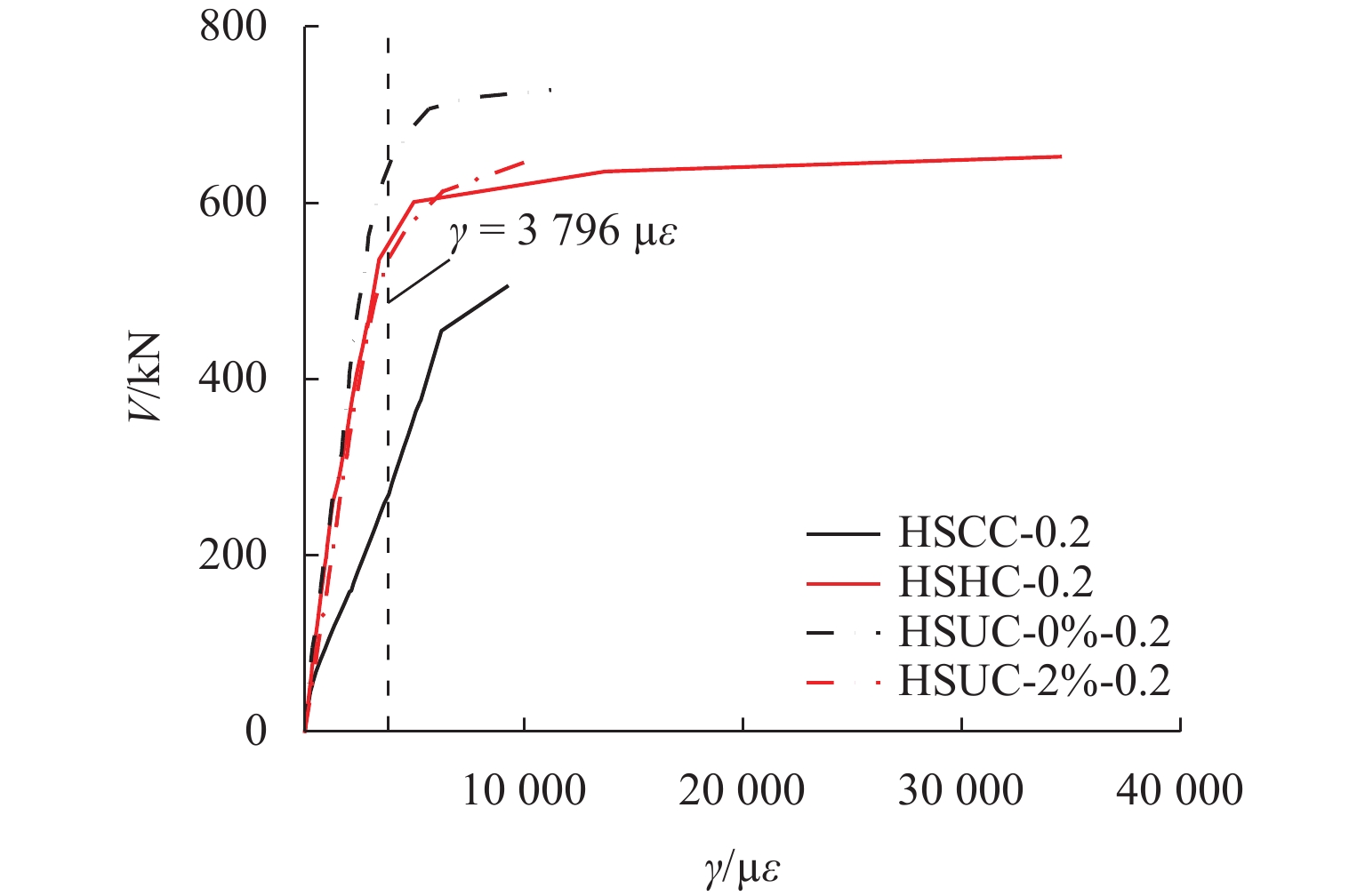
2.4 剪力-剪应变曲线
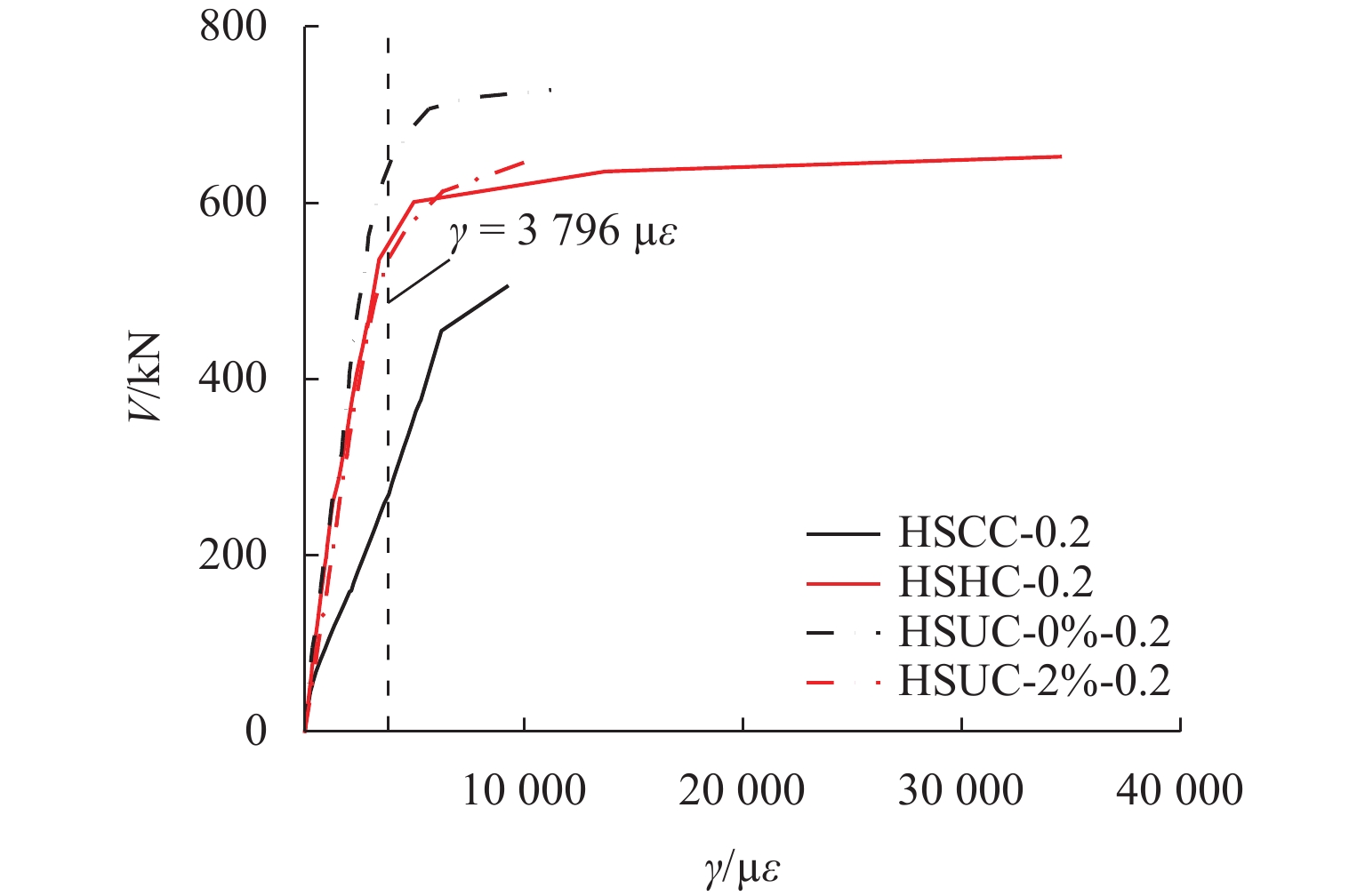
试验结果表明,所有试样2个剪跨区的变化趋势一致. 本文以剪跨比为0.2的试件为例,取5号应变花处的数据绘制剪力-剪应变(γ)关系曲线,如图10所示. 由图可知:达到峰值荷载时,所有试件的钢材均已屈服. 曲线可大致分为弹性、非弹性2个阶段. 达到极限承载力的70%左右时,钢管逐渐屈服、试件进入非弹性阶段.
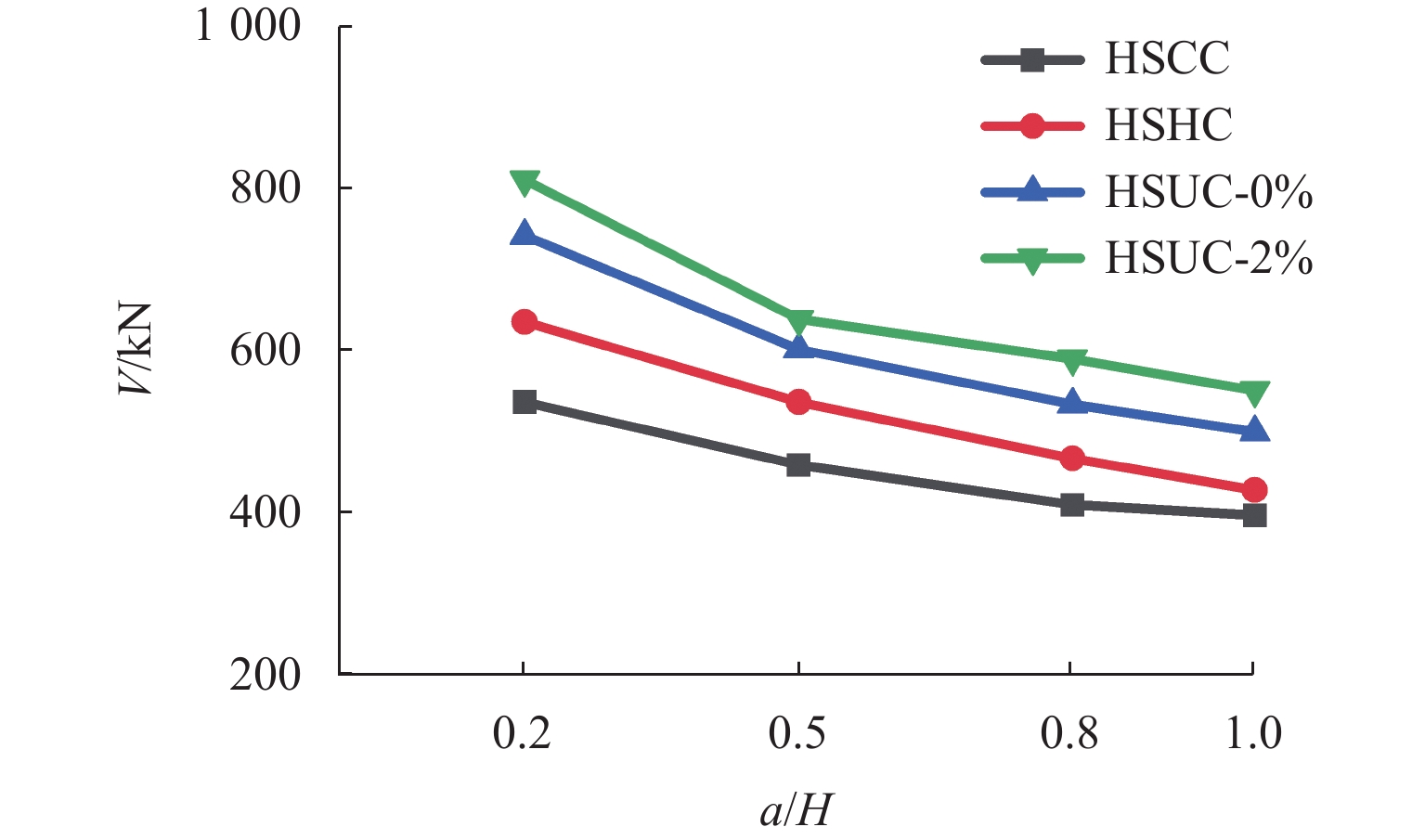
2.5 抗剪强度
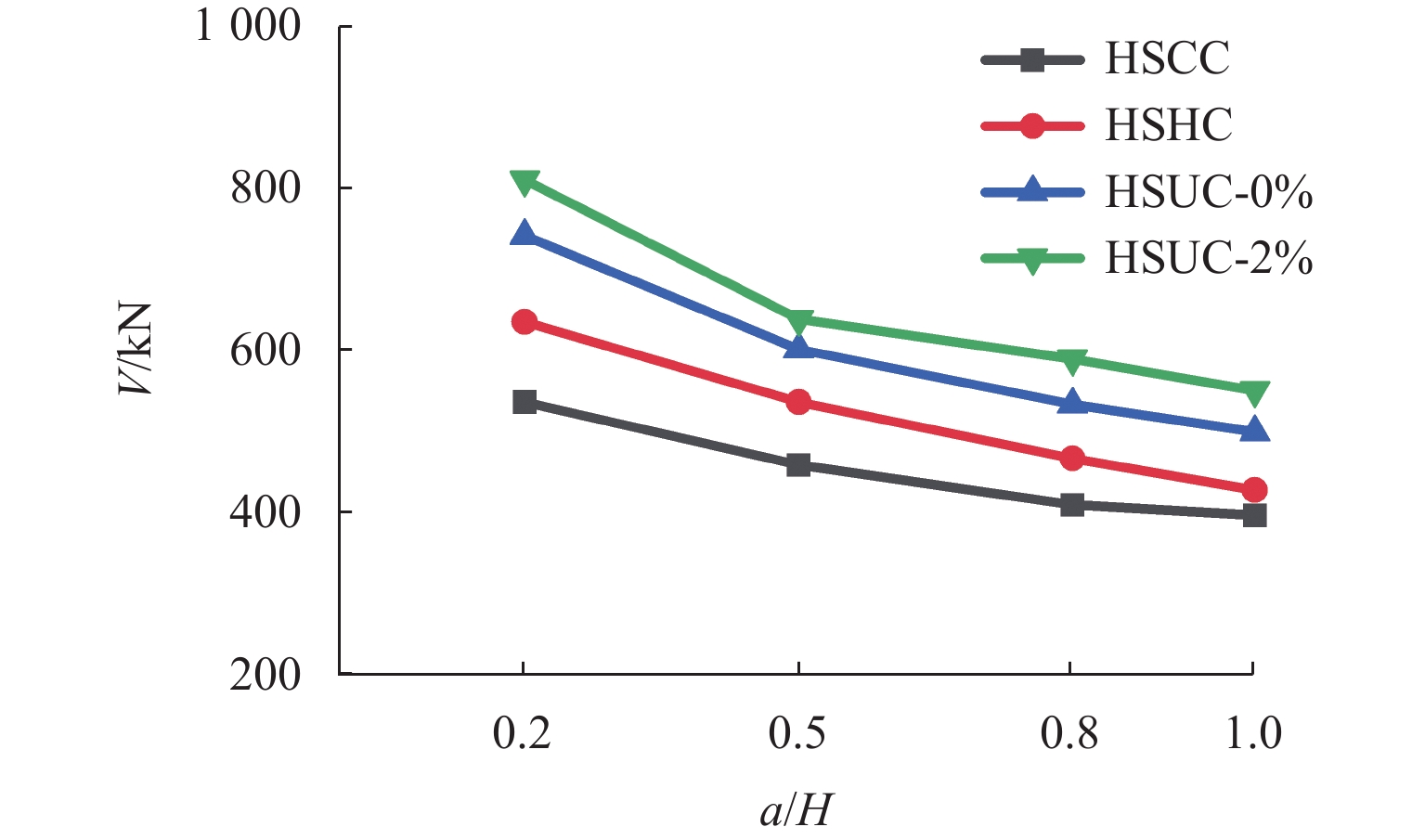
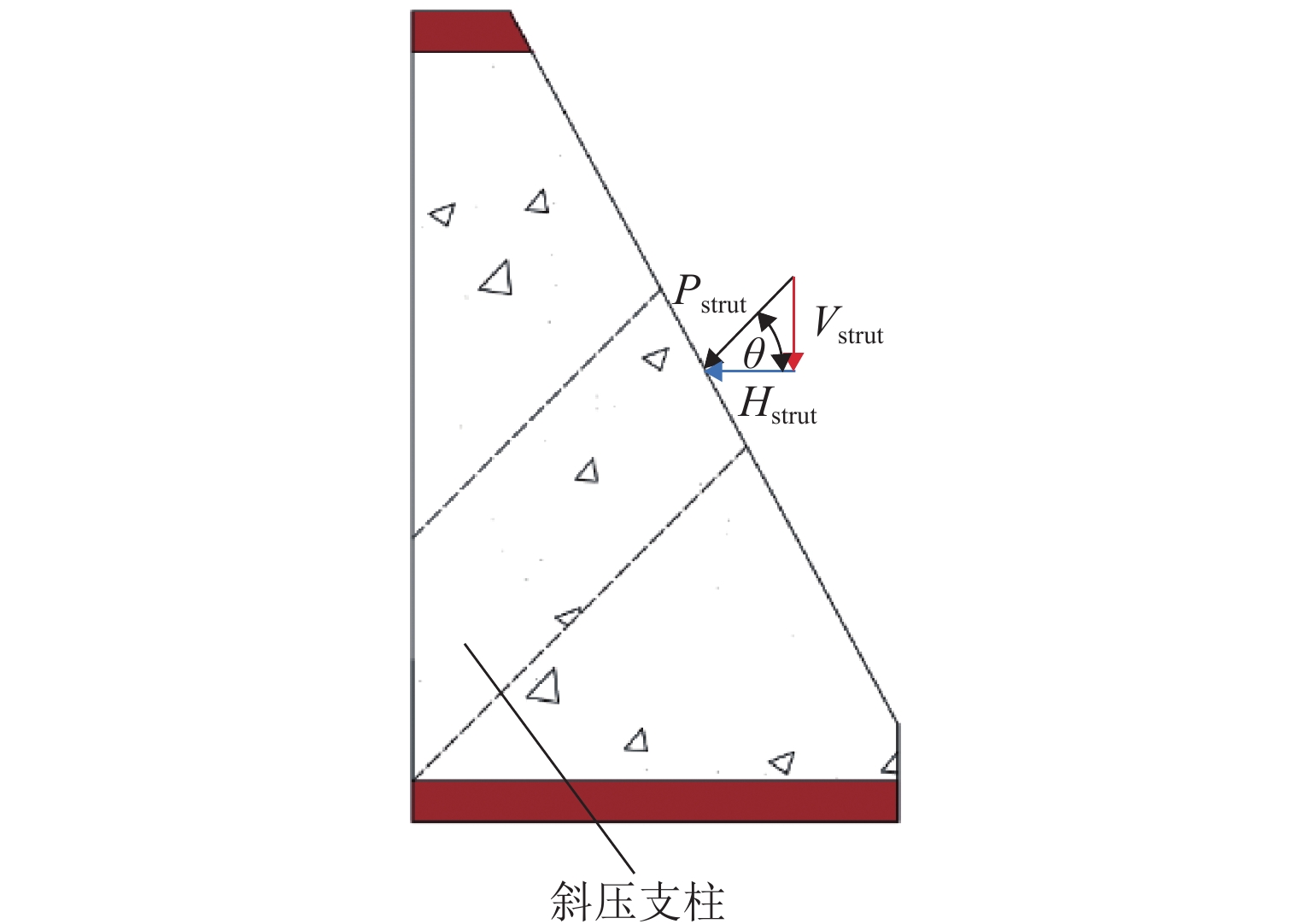
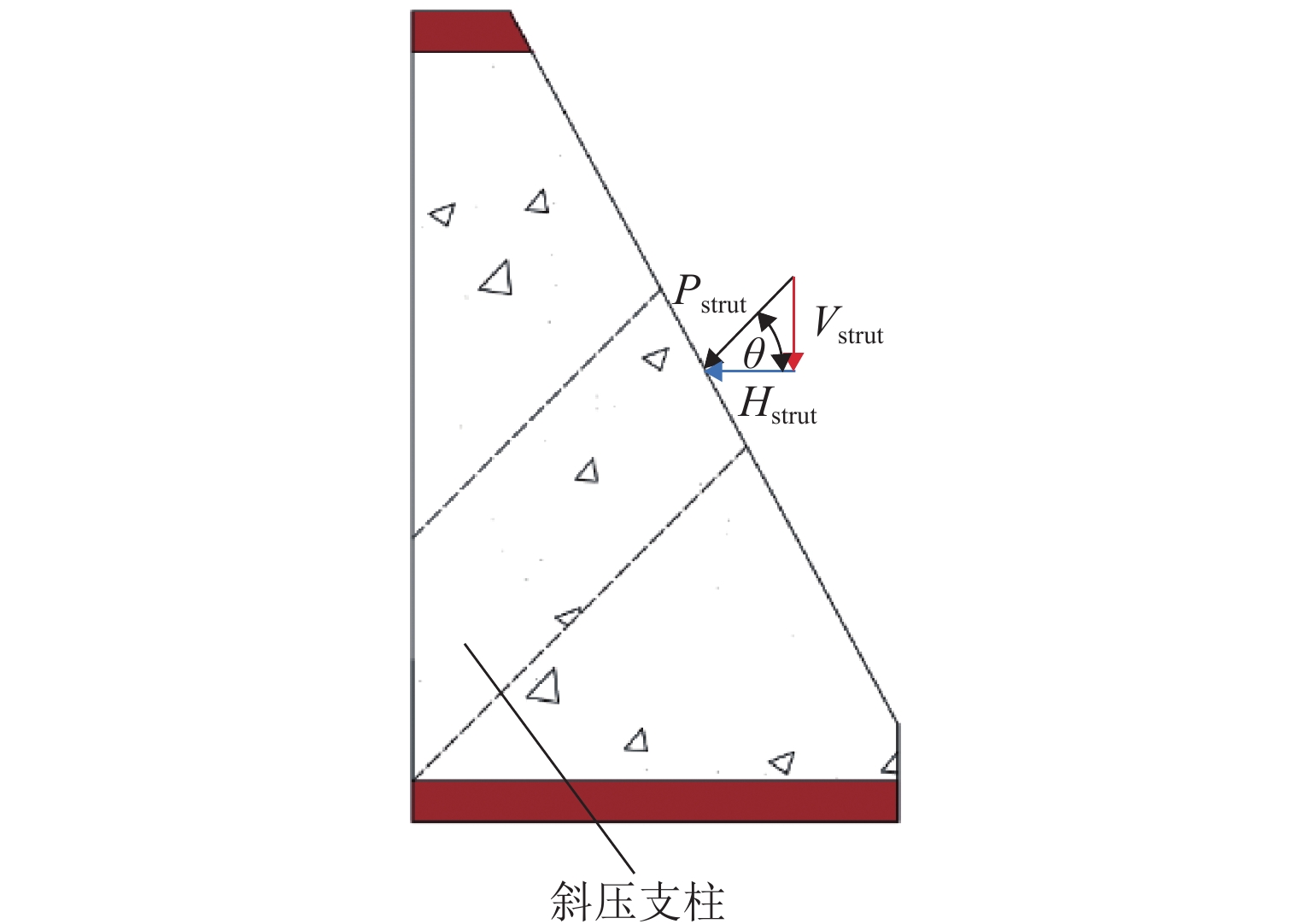
图11为不同研究参数对抗剪强度的影响,其中包括混凝土强度和剪跨比. 由图11可知:1) 在剪跨比相同的情况下,提高核心混凝土强度,抗剪强度显著提升. 例如,与试件HSUC-2%-0.2相比,试件HSUC-0%-0.2、HSHC-0.2和HSCC-0.2的抗剪强度分别降低了8.5%、27.6%和51.2%. 2) 在混凝土强度相同的情况下,增大剪跨比,抗剪强度显著降低. 例如,当剪跨比从0.2增加至1.0时,HSUC-2%、HSUC-0%、HSHC和HSCC系列试件的抗剪强度分别降低了32.2%、32.7%、32.8%和26.1%. 这是因为试件的抗剪强度主要由外部钢管和核心混凝土两部分提供. 其中,外部钢管的抗剪强度主要由腹板提供,而混凝土的抗剪强度主要由斜压柱提供[24],该斜压柱的形状为有角度(θ)的立方体,如图12所示. 图中,Pstrut为施加的荷载. 正如2.2节所分析的,剪跨比是影响θ的主要因素,且随剪跨比的增加而减少. 由图12可知:当试件受到剪切变形时,对Pstrut进行分解,会产生一个水平力Hstrut和竖向力Vstrut (Vstrut为核心混凝土提供的抗剪强度);剪跨比越小,θ越大,导致Vstrut增大,核心混凝土提供的抗剪强度增大;混凝土抗压强度越高,Pstrut越大,导致Vstrut增大,核心混凝土提供的抗剪强度增大.
综上,剪跨比和混凝土强度对高强方钢管混凝土试件的抗剪强度影响显著. 在混凝土强度相同的情况下,剪跨比较大的试件抗剪强度较低;在剪跨比相同的情况下,混凝土强度越高,抗剪强度越高.
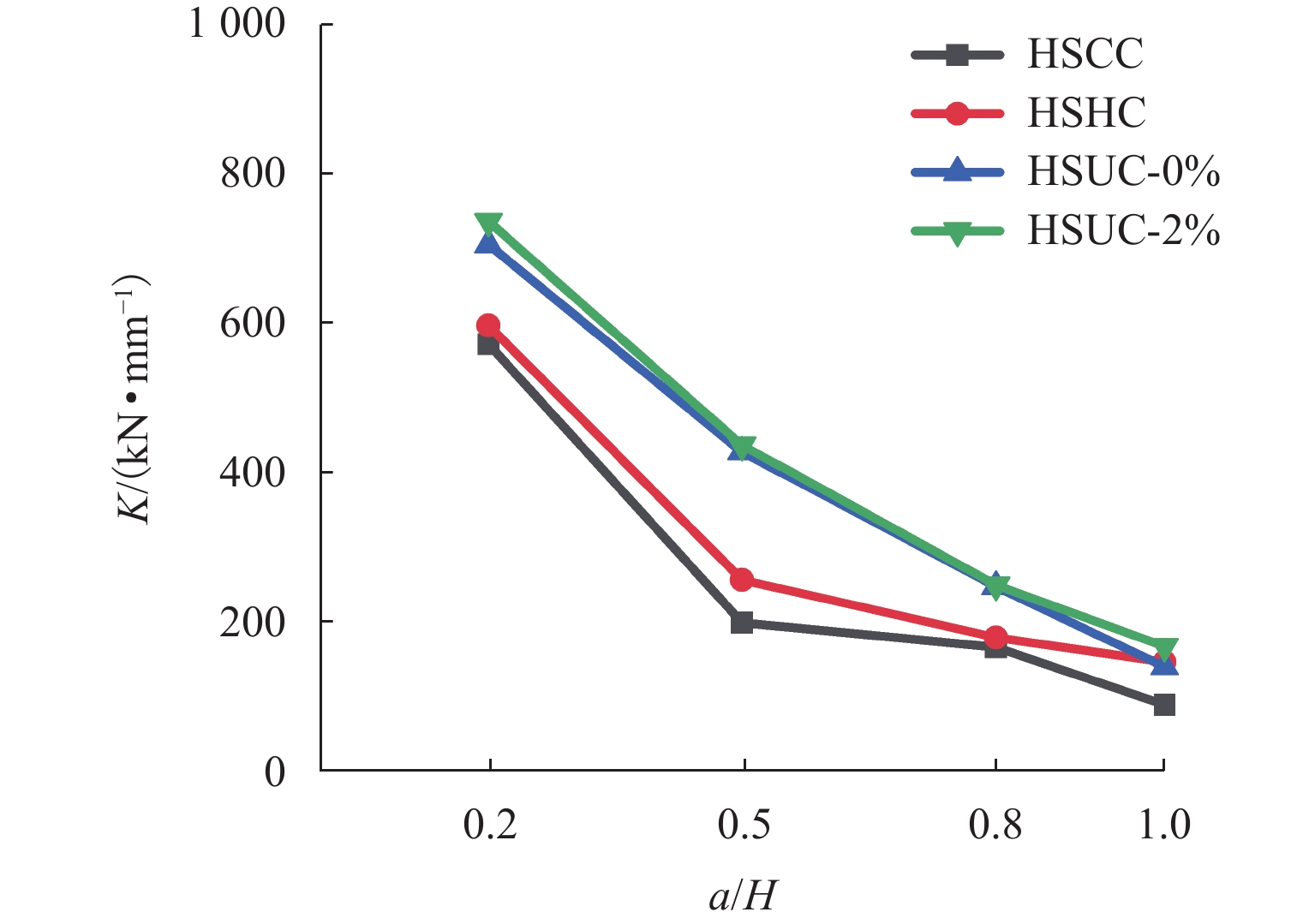
2.6 抗剪刚度
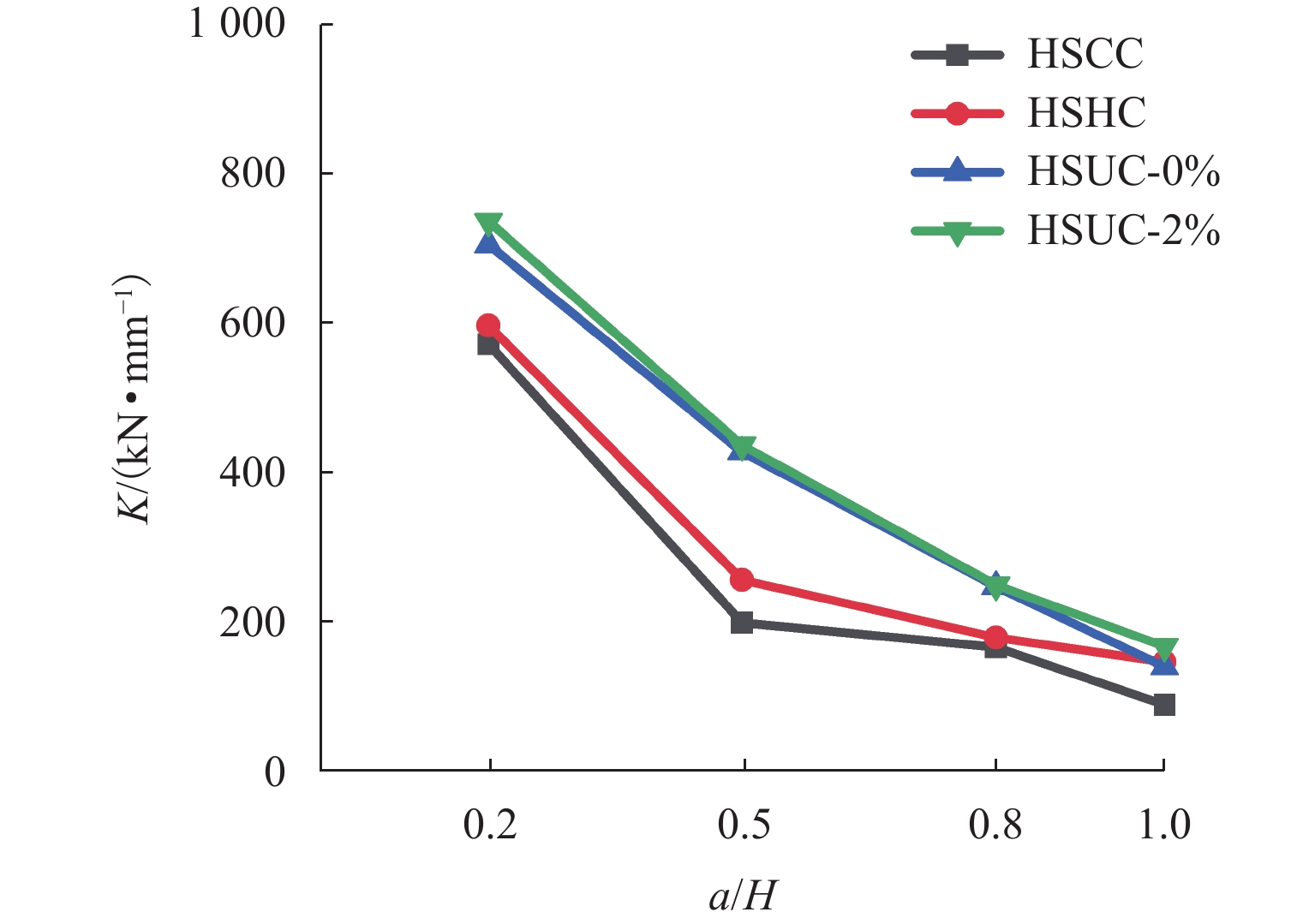
抗剪刚度K定义为达到极限抗剪强度前0.7Vexp对应的斜率. 图13为不同研究参数对抗剪刚度的影响,其中包括混凝土强度和剪跨比. 由图13可知:1) 在剪跨比相同的情况下,提高混凝土强度,抗剪刚度增强. 如与试件HSUC-2%-0.2相比,试件HSUC-0%-0.2、HSHC-0.2和HSCC-0.2的抗剪刚度分别降低了4.2%、23.2%和28.5%. 2) 在混凝土强度相同的情况下,增大剪跨比,抗剪刚度降低,当剪跨比从0.2增加至1.0时, HSUC-2%、HSUC-0%、HSHC以及HSCC系列试件的抗剪刚度分别降低了77.1%、80%、75.3%和84.2%. 可以看出,混凝土强度对抗剪刚度有显著影响,但是影响抗剪刚度的关键参数为剪跨比. 具体表现为:在混凝土强度相同的情况下,剪跨比较大的试件抗剪刚度较低;在剪跨比相同的情况下,混凝土强度越高,抗剪刚度越高.
3. 现有公式评估
目前,用于计算钢管混凝土抗剪承载力的规范主要包括我国钢管混凝土结构技术规程[20]、美国路桥设计规范[21]、欧洲规范[22]和华盛顿桥梁设计规范[23]等.
钢管混凝土结构技术规程[20]推荐用式(1)计算抗剪强度 (Vu),同时考虑轴压比和剪跨比,计算公式如式(2).
Vu=(V0 + 0.1N1)(1−0.45√aH), (1) V0=Acfcv + Asfsy, (2) 式中:N1为与Vu对应的轴向压力设计值;V0为钢管混凝土单肢柱受纯剪时的抗剪承载力设计值;Ac为钢管内核心混凝土的横截面面积;As为钢管的横截面面积;fcv=2ft≈0.2fc,为混凝土的抗剪强度,ft和 fc分别为钢管内核心混凝土的抗拉和抗压强度设计值;fsy=fy√3≈0.6fy,为钢管的抗剪强度.
整理可得
V0=0.2Acfc(1 + 3δ), (3) 式中:δ为钢管混凝土的套箍指标,δ=Asfy/Acfc.
文献[21]建议用式(4)、(5)分别计算Vs和Vc,两者相加即为钢管混凝土的抗剪承载力VA.
Vs=0.5FcrAs, (4) Fcr1=1.60Es√LvB(Bt)54⩽ (4a) {{F}}_{{{\mathrm{cr}}}\text{2}}\text=\frac{\text{0.78}{{E}}_{{{\mathrm{s}}}}}{{\left(\dfrac{{B}}{{t}}\right)}^{\tfrac{\text{3}}{\text{2}}}}\leqslant\text{0.58}{{F}}_{{{\mathrm{y}}}} \text{,} (4b) {{V}}_{{{\mathrm{c}}}}\text{=0.012 86}{\beta }{{A}}_{{{\mathrm{c}}}}\sqrt{{{f}}_{\text{c1}}} , (5) 式中:Fcr为剪切屈曲临界应力,取式(4a)和(4b)的最大值;Lv为最大剪力点到零剪力点之间的距离;B和t为钢管的宽度和厚度;β为系数,取2.0.
考虑到轴压力的影响,文献[22]建议用式(6)和式(7)分别计算Vs和Vc,两者相加即为钢管混凝土的抗剪承载力VE.
{{V}}_{{{\mathrm{s}}}}\text=\frac{{{A}}_{{{\mathrm{v}}}} {{{f}}_{{{\mathrm{yd}}}}}/{\sqrt{\text{3}}}}{{{\gamma }}_{{{\mathrm{M}}}{0}}} \text{,} (6) {{A}}_{{{\mathrm{v}}}}={{A}}_{{{\mathrm{s}}}}{H}\left({B}\text{ + }{H}\right) \text{,} (6a) {{V}}_{{{\mathrm{c}}}}{=0.85}\nu {{f}}_{{{\mathrm{cd}}}}{{A}}_{{{\mathrm{c}}}}\sin\;{\varphi } \text{,} (7) {{A}}_{{{\mathrm{c}}}}\text{=0.8}\left({b}-{{t}}_{{{\mathrm{w}}}}\right)\left({H}{-2}{{t}}_{{{\mathrm{f}}}}\right)\cos\;{\varphi } \text{,} (7a) {v}\text{=0.55}\left[\text{1 + 2}{{N}_{{{\mathrm{ed}}}}}/{{N}_{{{\mathrm{pl,rd}}}}}\right]\leqslant\text{1.1}\text{,} (7b) 式中:fyd为钢材屈服应力;fcd为混凝土抗压圆柱体强度;φ=arctan[(H-2tf)/z],z为力臂;Av为钢管的剪切面积;γM0为局部系数,取1.0;b为填充混凝土的宽度;v为考虑了轴压力影响的折减系数;Ned为施加的轴压力;Npl,rd为塑性阻力;tw和tf分别为钢管腹板和翼板的厚度.
文献[23]考虑了钢筋对抗剪强度的影响,建议采用式(8)来计算抗剪承载力.
{{V}}_{{{\mathrm{W}}}}{=2}{{V}}_{{{\mathrm{s}}}}{ + }{{V}}_{{{\mathrm{sr}}}}{ + }{\eta }{{V}}_{{{\mathrm{c}}}} {,} (8) {{V}}_{{{\mathrm{s}}}}{=0.6}{{F}}_{{{\mathrm{y}}}}\left({0.5}{{A}}_{{{\mathrm{s}}}}\right) {,} (8a) {{V}}_{{{\mathrm{sr}}}}{=0.6}{{F}}_{{{\mathrm{yr}}}}\left({0.5}{{A}}_{{{\mathrm{sr}}}}\right) {,} (8b) {\eta }{=5}\left({1 + 5}{{p}}/{{{p}}_{{0}}}\right){\leqslant 10} {,} (8c) {{V}}_{{{\mathrm{c}}}}\text{=0.012 86}{{A}}_{{{\mathrm{c}}}}\sqrt{{{f}}_{\text{c1}}}, (8d) 式中:p为施加的荷载, p0 为钢管混凝土组合构件的名义抗压强度; Vsr、Asr和Fyr分别为钢筋的抗剪强度、截面面积和屈服应力.
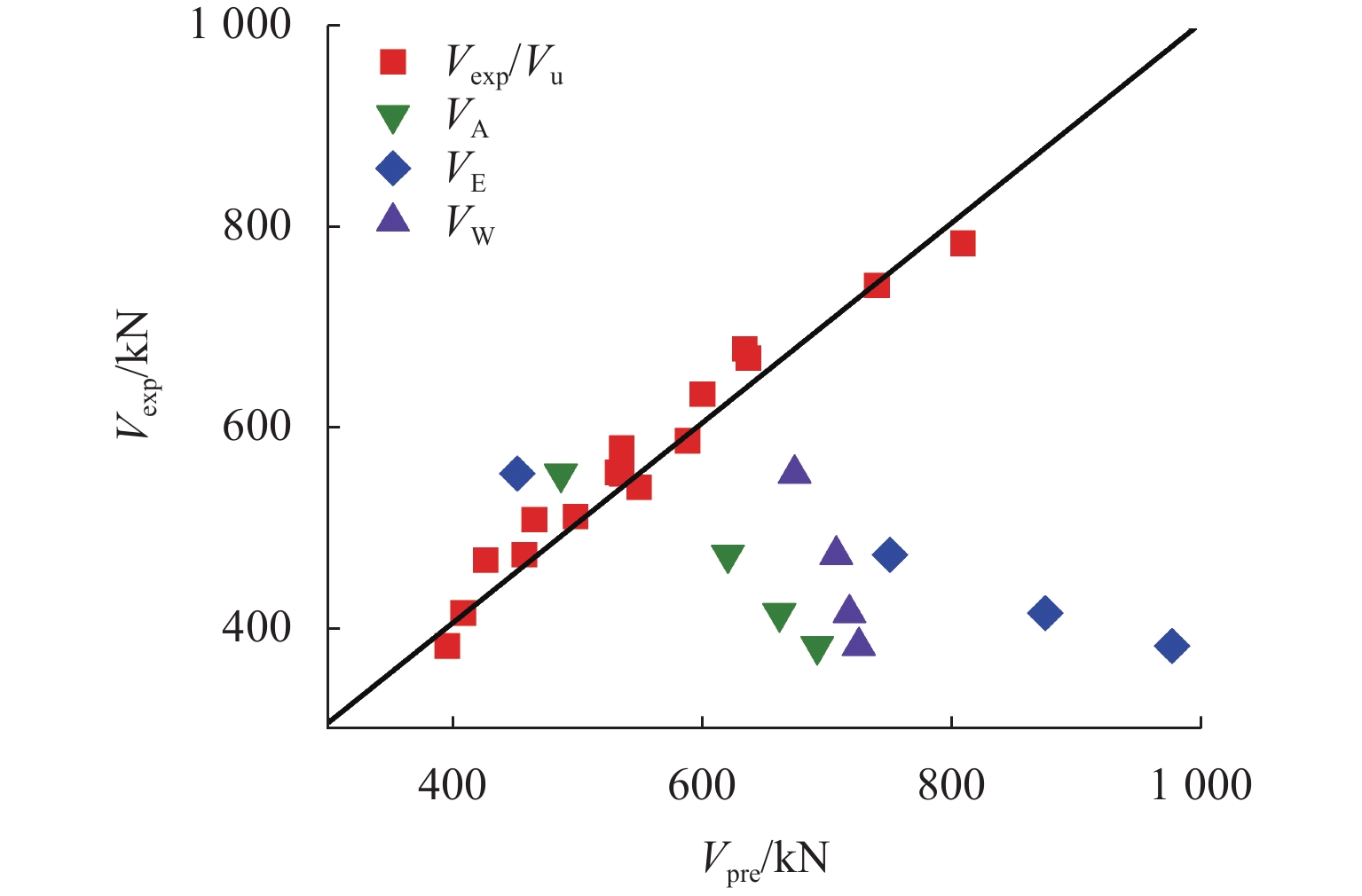
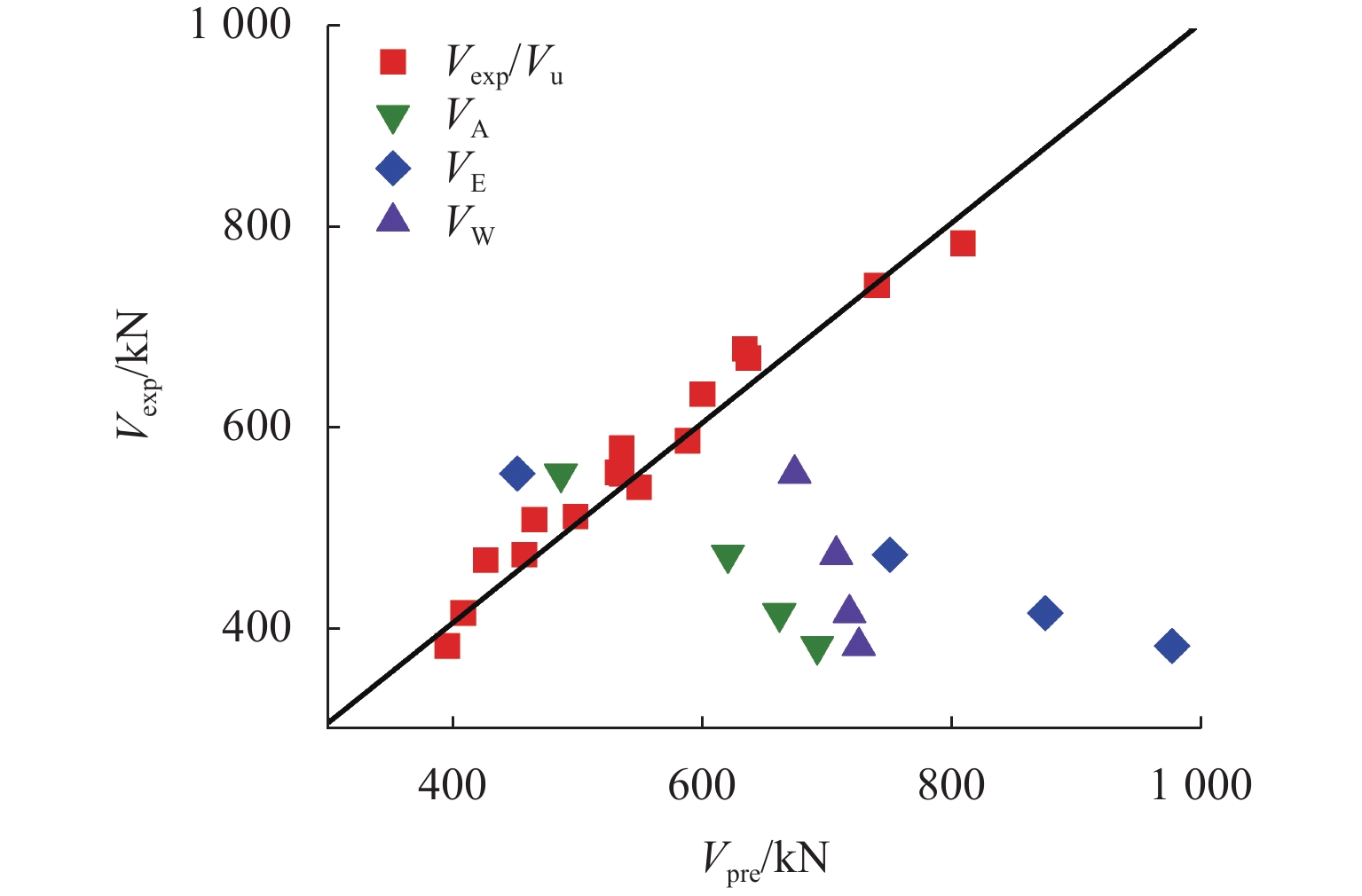
将试件参数代入式(1)~(8),所得计算值与试验值比较结果见图14和表4. 图中:Vpre为抗剪强度计算值. 由图14和表4可知:文献[20]的Vexp/Vu均值为0.97,方差为0.03,精度最高,离散性较小,且考虑了剪跨比的影响,推荐采用.
表 4 试验值与计算值比较Table 4. Comparison between the tested and calculated values试件编号 Vexp/kN 文献[20] 文献[21] 文献[22] 文献[23] Vu/kN Vexp/Vu VA/kN Vexp/VA VE/kN Vexp/VE VW/kN Vexp/VW HSCC-0.2 536 553.5 0.97 487.2 1.10 452.3 1.19 675.0 0.79 HSCC-0.5 458 472.4 0.97 487.2 0.94 452.3 1.01 675.0 0.68 HSCC-0.8 409 414.0 0.99 487.2 0.84 452.3 0.90 675.0 0.61 HSCC-1.0 396 381.1 1.01 487.2 0.81 452.3 0.88 675.0 0.59 HSHC-0.2 635 678.0 0.94 621.3 1.02 751.4 0.85 708.5 0.90 HSHC-0.5 536 578.8 0.93 621.3 0.86 751.4 0.71 708.5 0.76 HSHC-0.8 466 507.2 0.92 621.3 0.75 751.4 0.62 708.5 0.66 HSHC-1.0 427 466.9 0.91 621.3 0.69 751.4 0.57 708.5 0.60 HSUC-0%-0.2 741 741.4 1.00 662.6 1.12 876.1 0.85 718.9 1.03 HSUC-0%-0.5 601 632.8 0.95 662.6 0.91 876.1 0.69 718.9 0.84 HSUC-0%-0.8 533 554.6 0.96 662.6 0.80 876.1 0.61 718.9 0.74 HSUC-0%-1.0 499 510.5 0.98 662.6 0.75 876.1 0.57 718.9 0.69 HSUC-2%-0.2 810 783.7 1.03 693.1 1.17 977.8 0.83 726.5 1.11 HSUC-2%-0.5 638 669.0 0.95 693.1 0.92 977.8 0.65 726.5 0.88 HSUC-2%-0.8 589 586.3 1.00 693.1 0.85 977.8 0.60 726.5 0.81 HSUC-2%-1.0 550 539.7 1.02 693.1 0.79 977.8 0.56 726.5 0.76 均值 0.97 0.90 0.75 0.78 方差 0.03 0.14 0.18 0.15 4. 结 论
1) 与普通钢管混凝土试件一致,剪跨比是高强方钢管混凝土试件破坏模式的主要控制参数. 当剪跨比为0.2或0.5时,发生剪切破坏;当剪跨比为0.8或1.0时,发生弯剪破坏. 此外,提高混凝土强度可有效延缓钢管局部屈曲并降低核心混凝土破坏程度.
2) 对于高强钢管混凝土试件,核心混凝土主斜裂缝形成的倾角θ随剪跨比增大而减小,混凝土强度对其无明显影响;剪跨比和混凝土强度是影响其抗剪性能的主要因素,降低剪跨比和提高混凝土强度可显著增强试件的抗剪强度和刚度.
3) 混凝土强度相同时,剪跨比较大的试件具有更好的变形能力和更长的非弹性工作阶段,但弹性刚度较低;剪跨比相同时,混凝土强度高的试件表现出较差的变形能力和较短的非弹性工作阶段,但弹性刚度较大.
4) 钢管混凝土结构技术规程提出的计算公式对高强方钢管混凝土构件同样适用,可用于计算其抗剪强度.
-
表 1 试件主要参数
Table 1. Main parameters of specimens
序号 试件编号 L/mm B/mm H/mm \rho /% f_{\mathrm{y}} /MPa f_{{\mathrm{c}}1} /MPa t/mm E_{\mathrm{s}} /GPa E_{\mathrm{c}} /GPa 1 HSCC-0.2 1000 120 120 747 30 3 206 32.7 2 HSCC-0.5 1000 120 120 747 30 3 206 32.7 3 HSCC-0.8 1000 120 120 747 30 3 206 32.7 4 HSCC-1.0 1000 120 120 747 30 3 206 32.7 5 HSHC-0.2 1000 120 120 782 90 3 206 43.4 6 HSHC-0.5 1000 120 120 782 90 3 206 43.4 7 HSHC-0.8 1000 120 120 782 90 3 206 43.4 8 HSHC-1.0 1000 120 120 782 90 3 206 43.4 9 HSUC-0%-0.2 1000 120 120 747 115 3 206 41.9 10 HSUC-0%-0.5 1000 120 120 747 115 3 206 41.9 11 HSUC-0%-0.8 1000 120 120 747 115 3 206 41.9 12 HSUC-0%-1.0 1000 120 120 747 115 3 206 41.9 13 HSUC-2%-0.2 1000 120 120 2 775 135.4 3 206 44.8 14 HSUC-2%-0.5 1000 120 120 2 775 135.4 3 206 44.8 15 HSUC-2%-0.8 1000 120 120 2 775 135.4 3 206 44.8 16 HSUC-2%-1.0 1000 120 120 2 775 135.4 3 206 44.8 表 2 混凝土配合比
Table 2. Concrete mix proportions
kg/m3 混凝土类别 水 水泥 硅灰 粗骨料 细骨料 减水剂 钢纤维体积掺量/% 普通(C30) 180.0 370.0 1042.0 766.0 7.40 高强(C90) 156.8 579.4 102.2 1050.8 617.2 15.48 UHPC (无钢纤维) 221.7 852.5 255.7 920.7 80.1 21.30 UHPC (2% 钢纤维) 221.7 852.5 255.7 920.7 80.1 21.30 2 表 3 试验结果
Table 3. Test results
试件编号 Vexp/
kN\varDelta_{\mathrm{y}} /
mm\varDelta_{\mathrm{u}} /
mmK/
(kN·mm−1)破坏模式 HSCC-0.2 536 0.9 9.5 573 剪切破坏 HSCC-0.5 458 2.3 28.4 201 剪切破坏 HSCC-0.8 409 2.4 29.7 168 弯剪破坏 HSCC-1.0 396 4.4 >30.0 91 弯剪破坏 HSHC-0.2 635 1.1 7.2 598 剪切破坏 HSHC-0.5 536 2.1 22.6 258 剪切破坏 HSHC-0.8 466 2.5 27.7 181 弯剪破坏 HSHC-1.0 427 2.9 >30.0 148 弯剪破坏 HSUC-0%-0.2 741 1.1 14.5 706 剪切破坏 HSUC-0%-0.5 601 1.4 27.8 429 剪切破坏 HSUC-0%-0.8 533 2.1 21.2 249 弯剪破坏 HSUC-0%-1.0 499 3.5 23.5 142 弯剪破坏 HSUC-2%-0.2 810 1.1 6.1 737 剪切破坏 HSUC-2%-0.5 638 1.6 14.2 438 剪切破坏 HSUC-2%-0.8 589 2.3 22.4 251 弯剪破坏 HSUC-2%-1.0 550 3.3 23.3 169 弯剪破坏 表 4 试验值与计算值比较
Table 4. Comparison between the tested and calculated values
试件编号 Vexp/kN 文献[20] 文献[21] 文献[22] 文献[23] Vu/kN Vexp/Vu VA/kN Vexp/VA VE/kN Vexp/VE VW/kN Vexp/VW HSCC-0.2 536 553.5 0.97 487.2 1.10 452.3 1.19 675.0 0.79 HSCC-0.5 458 472.4 0.97 487.2 0.94 452.3 1.01 675.0 0.68 HSCC-0.8 409 414.0 0.99 487.2 0.84 452.3 0.90 675.0 0.61 HSCC-1.0 396 381.1 1.01 487.2 0.81 452.3 0.88 675.0 0.59 HSHC-0.2 635 678.0 0.94 621.3 1.02 751.4 0.85 708.5 0.90 HSHC-0.5 536 578.8 0.93 621.3 0.86 751.4 0.71 708.5 0.76 HSHC-0.8 466 507.2 0.92 621.3 0.75 751.4 0.62 708.5 0.66 HSHC-1.0 427 466.9 0.91 621.3 0.69 751.4 0.57 708.5 0.60 HSUC-0%-0.2 741 741.4 1.00 662.6 1.12 876.1 0.85 718.9 1.03 HSUC-0%-0.5 601 632.8 0.95 662.6 0.91 876.1 0.69 718.9 0.84 HSUC-0%-0.8 533 554.6 0.96 662.6 0.80 876.1 0.61 718.9 0.74 HSUC-0%-1.0 499 510.5 0.98 662.6 0.75 876.1 0.57 718.9 0.69 HSUC-2%-0.2 810 783.7 1.03 693.1 1.17 977.8 0.83 726.5 1.11 HSUC-2%-0.5 638 669.0 0.95 693.1 0.92 977.8 0.65 726.5 0.88 HSUC-2%-0.8 589 586.3 1.00 693.1 0.85 977.8 0.60 726.5 0.81 HSUC-2%-1.0 550 539.7 1.02 693.1 0.79 977.8 0.56 726.5 0.76 均值 0.97 0.90 0.75 0.78 方差 0.03 0.14 0.18 0.15 -
[1] 韩林海. 钢管混凝土结构:理论与实践[M]. 2版. 北京:科学出版社,2007. [2] LAI Z C, VARMA A H, GRIFFIS L G. Analysis and design of noncompact and slender CFT beam-columns[J]. Journal of Structural Engineering, 2016, 142(1): 04015097.1-04015097.14. [3] WANG Y, LIN H, LAI Z C, et al. Flexural behavior of high-strength rectangular concrete-filled steel tube members[J]. Journal of Structural Engineering, 2022, 148(1): 4021230.1-4021230.15. [4] WANG F Y, ZHAO O, YOUNG B. Testing and numerical modelling of S960 ultra-high strength steel angle and channel section stub columns[J]. Engineering Structures, 2020, 204: 109902.1-109902.12. [5] RICHARD P, CHEYREZY M. H. Reactive powder concretes with high ductility and 200-800 MPa compressive strength[J]. Special Publications, 1994, 144(24): 507-518. [6] 管民生,黄献奇,杜宏彪,等. 矩形钢管高强混凝土框架抗震性能分析[J]. 西南交通大学学报,2019,54(3): 483-491. doi: 10.3969/j.issn.0258-2724.20170809GUAN Minsheng, HUANG Xianqi, DU Hongbiao, et al. Seismic behavior of rectangular high-strength concrete-filled steel tube frame structure[J]. Journal of Southwest Jiaotong University, 2019, 54(3): 483-491. doi: 10.3969/j.issn.0258-2724.20170809 [7] YE Y, HAN L H, TAO Z, et al. Experimental behaviour of concrete-filled steel tubular members under lateral shear loads[J]. Journal of Constructional Steel Research, 2016, 122: 226-237. doi: 10.1016/j.jcsr.2016.03.012 [8] 黄勇,陈伟刚,段莉. 钢管混凝土短柱(剪力键)受剪性能试验研究[J]. 建筑结构学报,2011,32(12): 178-185.HUANG Yong, CHEN Weigang, DUAN Li. Experimental study on shear resistance performance of concrete filled steel tube shear block stub columns[J]. Journal of Building Structures, 2011, 32(12): 178-185. [9] 李志强,陈以一,王伟. 矩形钢管混凝土中短柱弯-剪性能试验研究[J]. 建筑结构学报,2015,36(7): 1-9.LI Zhiqiang, CHEN Yiyi, WANG Wei. Experimental research on bending and shearing behavior of middle and short concrete filled steel tubular columns[J]. Journal of Building Structures, 2015, 36(7): 1-9. [10] 史艳莉,周绪红,鲜威,等. 无端板矩形钢管混凝土构件基本剪切性能研究[J]. 工程力学,2018,35(12): 25-33.SHI Yanli, ZHOU Xuhong, XIAN Wei, et al. Research on basic shear performance of concrete filled rectangular steel tubular members without end-plate[J]. Engineering Mechanics, 2018, 35(12): 25-33. [11] 丁威. 矩形钢管混凝土双剪截面研究及其工程应用[D]. 广东:广东工业大学,2019. [12] MANSOURI A. Shear strength of concrete-filled steel tubes based on experimental results[J]. Journal of Structural Engineering, 2020, 146(6): 04020097.1-04020097.14. [13] LEHMAN D, ROEDER C, HEID A, et al. Shear response of concrete filled tubes part 1: experiments[J]. Journal of Constructional Steel Research, 2018, 150: 528-540. doi: 10.1016/j.jcsr.2018.08.027 [14] LEHMAN D, ROEDER C, HEID A, et al. Shear response of concrete filled tubes part Ⅱ: analytical study[J]. Journal of Constructional Steel Research, 2019, 153: 169-178. doi: 10.1016/j.jcsr.2018.08.019 [15] 颜燕祥,徐礼华,蔡恒,等. 高强方钢管超高性能混凝土短柱轴压承载力计算方法研究[J]. 建筑结构学报,2019,40(12): 128-137.YAN Yanxiang, XU Lihua, CAI Heng, et al. Calculation methods of axial bearing capacity of short square UHPC filled high strength steel tubular columns[J]. Journal of Building Structures, 2019, 40(12): 128-137. [16] 操礼林,石启印,王震,等. 高强U形外包钢-混凝土组合梁受弯性能[J]. 西南交通大学学报,2014,49(1): 72-78. doi: 10.3969/j.issn.0258-2724.2014.01.012CAO Lilin, SHI Qiyin, WANG Zhen, et al. Bending performances of high-strength steel-encased composite beams[J]. Journal of Southwest Jiaotong University, 2014, 49(1): 72-78. doi: 10.3969/j.issn.0258-2724.2014.01.012 [17] YAN Y X, XU L H, LI B, et al. Axial behavior of ultra-high performance concrete (UHPC) filled stocky steel tubes with square sections[J]. Journal of Constructional Steel Research, 2019, 158: 417-428. doi: 10.1016/j.jcsr.2019.03.018 [18] HU J Y, HUANG Y F, LI W J, et al. Compressive behaviour of UHPC-filled square high-strength steel tube stub columns under eccentric loading[J]. Journal of Constructional Steel Research, 2022, 198: 107558.1-107558.15. [19] LAI Z C, ZHOU W S, WANG Y, et al. Flexural behavior and design of rectangular ultra-high performance concrete-filled high-strength steel tube members[J]. Engineering Structures, 2023, 286: 116125.1-116125.15. [20] 哈尔滨工业大学,中国建筑科学研究院. 钢管混凝土结构技术规程:CECS 28—2012[S]. 北京:中国计划出版社,2012. [21] American Association of State Highway and Transportation Officials (AASHTO). AASHTO LRFD bridge design specifications[S]. Washington, D. C.: AASHTO, 2014. [22] CEN. Design of composite steel and concrete structures part 1-1: general rules and rules for buildings: DS/EN 1994-1-1[S]. Belgium: [s.n.], 1994. [23] WSDOT. Bridge design manual LRFD[S]. Olympia: [s.n.], 2018. [24] KENARANGI H, BRUNEAU M. Shear strength of composite circular reinforced concrete-filled steel tubes[J]. Journal of Structural Engineering, 2020, 146(1): 04019180.1-04019180.15. -





 下载:
下载:















 下载:
下载: