Calculations for Transition Displacement and Design Optimization for Moveable Point Frog
-
摘要:
为减小可动心轨辙叉心轨/翼轨密贴区域不足位移、降低心轨牵引点转换力、提高辙叉直向平顺性,提出可动心轨辙叉的设计参数和关键部件优化设计方法. 以18号可动心辙叉最小轮缘槽宽度为优化目标,基于既有结构尺寸和有限元方法建立心轨转换计算模型,并采用逐次逼近法优化心轨转换位移曲线的设计方法;在满足辙叉直、侧向不同形位公差条件下,提出第2牵引点动程为50.7 mm的优化设计方案和直向开通状态下辙叉关键部件的结构设计方法. 研究表明:心轨计算转换位移与设计转换位移最大偏差为6.64 mm,位于弹性可弯中心;最小轮缘宽度计算值(90.7 mm)与实测平均值(90.9 mm)差异较小,满足车辆安全通过要求;第2牵引点动程较既有辙叉减小8.3 mm,减小了第2牵引点转换力.
Abstract:To reduce insufficient displacement in the contact area between the movable point frog’s point rail and wing rail, minimize transition force at the transition points of point rail, and improve the frog’s longitudinal smoothness, an optimization method for the design parameters and key components of movable point frogs was proposed. The minimum flangeway width of the No.18 movable point frog was selected as the optimization target. Based on the existing structural parameters and finite element method, a model for point rail transition calculation was established, and the method of successive approximation was used to optimize the design method of the transition displacement curve of the point rail. Under the different frog form and position tolerances for both straight/diverging lines, an optimized design was proposed with a second traction point stroke of 50.7 mm, along with the structural design scheme for key components of the frog in the straight-through state. The results show that the maximum deviation between calculated and designed point rail transition displacements is 6.64 mm, occurring at the elastic bending center. The computed minimum flangeway width (90.7 mm) closely matches the measured average value (90.9 mm), ensuring safe vehicle passage. Additionally, the second traction point stroke is reduced by 8.3 mm compared to existing frog designs, lowering the required transition force at the second traction point.
-
Key words:
- moveable point frog /
- finite element method /
- transition displacement /
- calculations /
- design
-
道岔是机车车辆从一股轨道转入或越过另一股轨道的线路设备,是铁路轨道的重要组成部分,也是线路上的薄弱环节[1]. 道岔由转辙器、导曲线和辙叉部分组成. 从结构上,辙叉可分为固定型辙叉和可动心轨辙叉. 可动心轨辙叉由翼轨、长心轨、短心轨、叉跟轨及联结零件组成. 按心轨跟端结构,可动心轨辙叉可分为单肢弹性可弯和双肢弹性可弯,单肢弹性可弯的长心轨跟端为固定端,短心轨跟端为斜接头;双肢弹性可弯的长、短心轨跟端均为固定端.
目前,我国时速200公里及以上道岔的辙叉均为可动心轨辙叉,12号、18号、30号辙叉采用单肢弹性可弯,42号及以上号码辙叉采用双肢弹性可弯. 可动心轨辙叉轨线连续可显著降低辙叉区轮轨的相互作用,提高行车平稳性[2],其通过心轨和不同侧翼轨密贴实现. 当列车顺向或逆向通过时,车轮由心轨转移至翼轨或由翼轨转移至心轨. 若心轨/翼轨密贴间隙过大,则将对列车安全通过产生威胁. 因此,我国铁路道岔技术条件[3,4]和相关规章[5-7]从制造和维护的角度对心轨/翼轨密贴间隙做出明确规定. 基于上述规定,王树国等[8] 对7个铁路局、20个车站的28组在役高速可动心轨道岔进行了检测,共检出23处扣分项,平均每组道岔0.82处,出现频次较高.
心轨/翼轨不密贴是心轨转换不足位移的一种表现形式. 国内外学者针对尖轨、心轨转换不足位移开展了大量研究:蔡小培等[9]基于ANSYS软件中的二维梁单元BEAM54建立了心轨有限元仿真模型,分析不同牵引点设置和摩擦系数对42号道岔心轨转换不足位移和扳动力的影响,并提出应对措施;曾瑞东等[10]采用BEAM54梁单元建立尖轨转换模型,研究跟端轨底加工对尖轨转换的影响;马晓川等[11]基于ANSYS中的BEAM3梁单元建立小号码道岔尖轨有限元模型,分析密贴刚度对尖轨转换特性的影响;司道林等[12]采用BEAM188单元建立50 kg/m钢轨9号道岔尖轨转换模型,并研究单点牵引时弹性可弯尖轨的转换特性;Čedomir等[13]采用有限元方法建立了18.5号可动心轨辙叉转换计算模型,分析心轨转换力. 上述研究侧重于控制尖轨、心轨的最大不足位移(位于最后一个牵引点至固定端之间)和降低转换力. 与最大不足位移相比,密贴区域的不足位移相对较小,目前尚未有控制心轨/翼轨密贴区域不足位移的研究成果.
心轨不足位移指转换后心轨实际位移和设计位移沿轨道横向的差值. 心轨不足位移的大小除与牵引点布置、滑床板摩擦系数、轨件活动段长度等因素有关以外[14],还与密贴线型(心轨设计位移曲线)设计有关. 鉴于既有可动心轨辙叉已在全路大量服役,且总体状态良好[15],本文不对辙叉主要结构进行调整,仅从密贴线型(设计位移曲线)的角度展开研究. 当前,设计位移曲线通过弹性可弯中心法确定[16],采用几何作图法确定心轨转换位移曲线,将弹性可弯中心前的心轨简化为刚体,但实际心轨为弹性体,转换时从尖端到固定端为连续变形,且心轨与翼轨密贴区域截面变化较为显著,因此,心轨实际转换位移曲线与设计转换位移曲线必然存在偏差.
鉴于此,本文以目前应用最多的18号可动心轨辙叉[17]为研究对象,基于由弹性可弯中心法确定的心轨结构参数,并采用实体单元建立有限元模型,提出心轨转换位移曲线计算方法和可动心轨辙叉关键部件的优化设计方法,拟减小心轨/翼轨密贴间隙、优化辙叉结构,为新一代可动心轨辙叉的设计提供参考依据.
1. 心轨转换位移曲线计算方法
基于有限元方法建立心轨转换计算模型,关键结构参数如表1所示. 模型按辙叉直向开通建立,长、短心轨采用实体单元进行模拟,充分考虑其机加工后复杂的结构特征,密度为
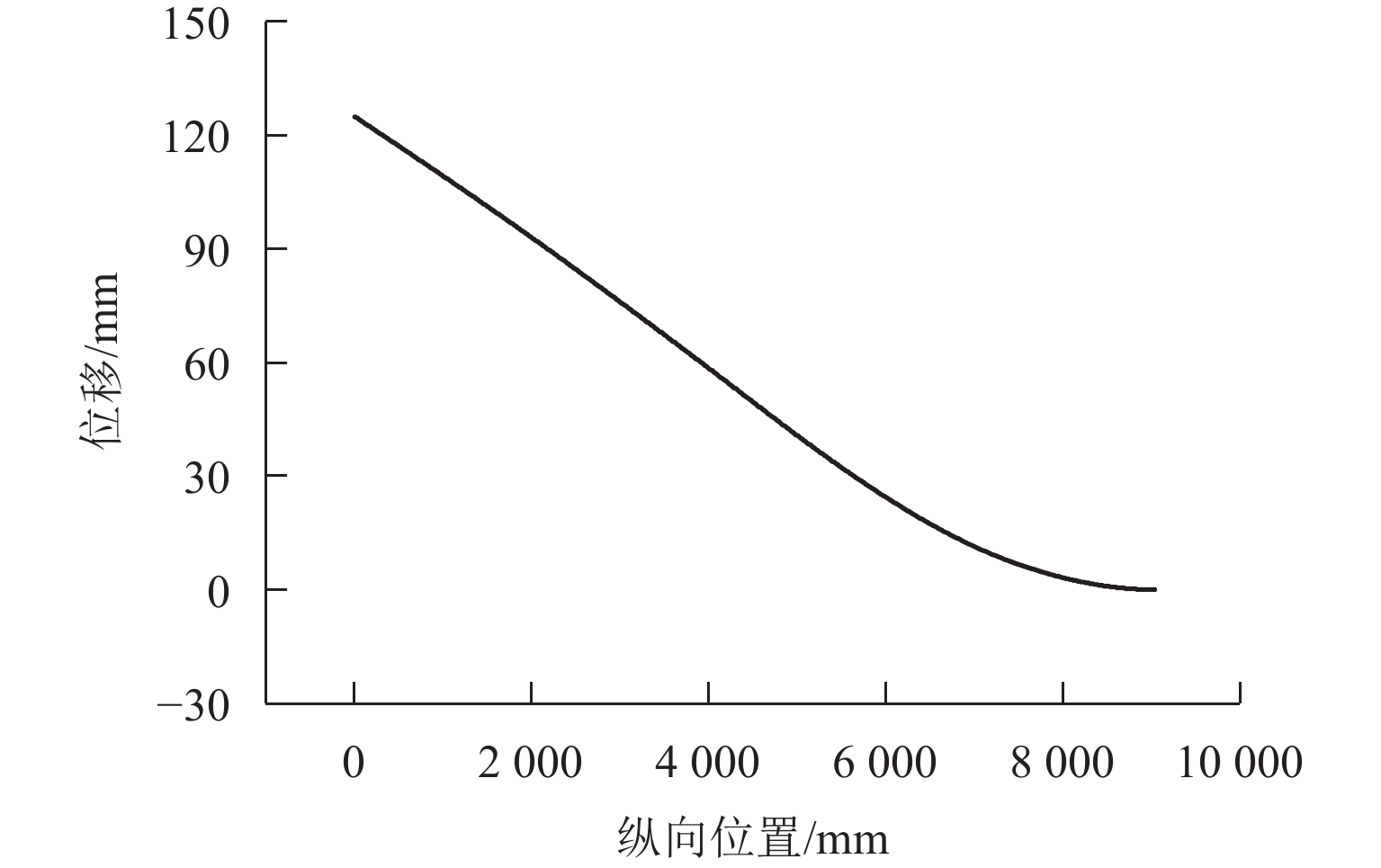
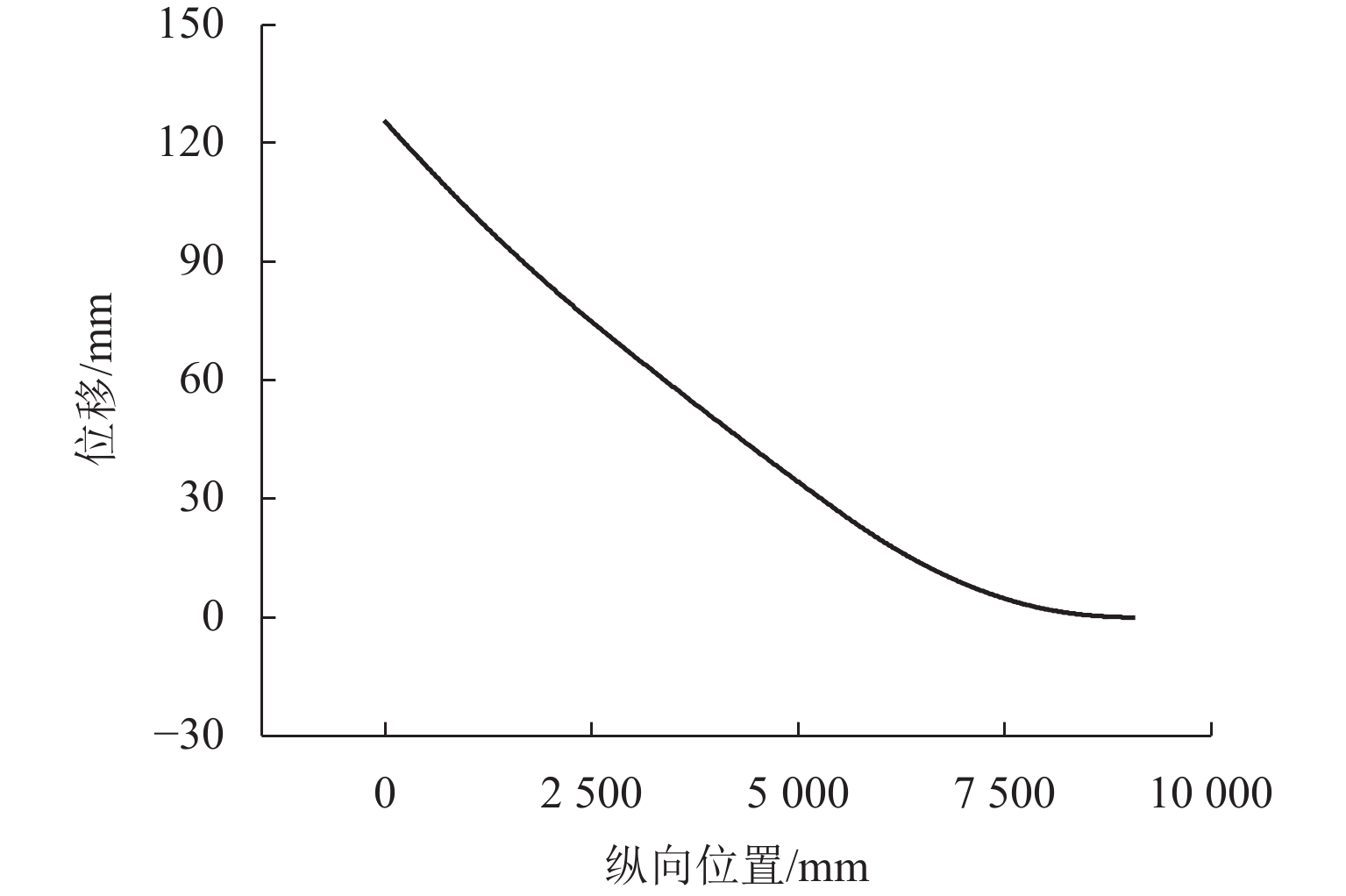
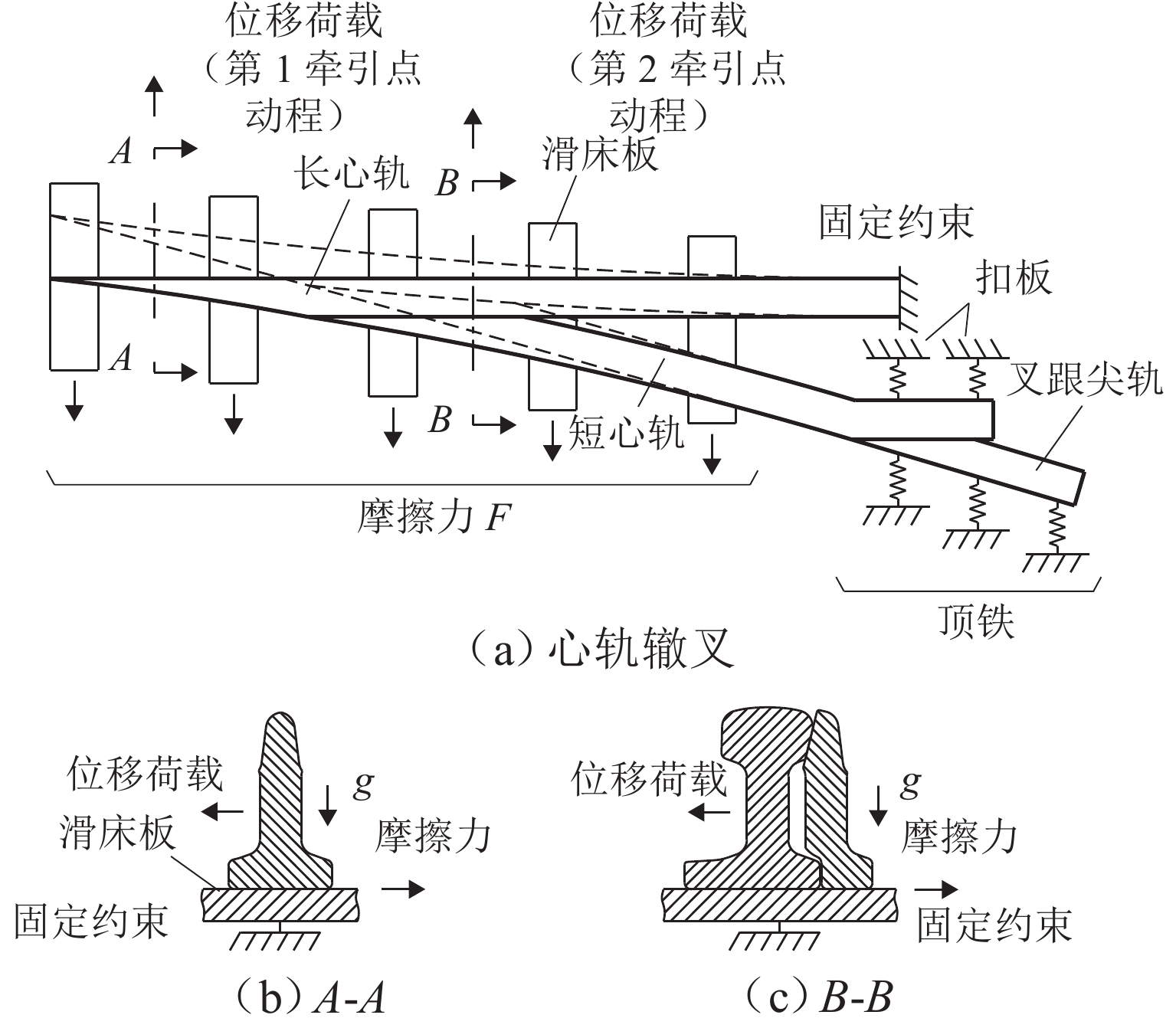
7850 kg/m3,弹性模量取为2.1 × 1011 Pa,泊松比取为0.3[18]. 对长心轨固定端施加完全约束. 在第1牵引点和第2牵引点分别施加对应牵引点动程的横向位移荷载. 在模型中设置沿y轴负方向的重力加速度,取值为9806.6 mm/s2,在长、短心轨和滑床板间设置摩擦接触,共26个接触对,摩擦系数为0.25[19]. 将长、短心轨轨头和轨底密贴段设置耦合约束,限制两者沿接触面切向和法向的位移. 扣板、顶铁支撑刚度为100 kN/mm[20],采用Combine14弹簧单元模拟,计算模型如图1所示. 图中:A-A、B-B分别为A、B处的截面,图1(a)中向下箭头均为摩擦力F.表 1 18号可动心轨辙叉关键结构参数Table 1. Key structure parameters for No. 18 movable point frog结构参数 取值/mm 长心轨轨头加工长度 2169 短心轨轨头加工长度 2075 短心轨长度 8215 长心轨可动段长度 9055 弹性可弯中心距心轨理论尖端距离 7505 咽喉宽度 125 距心轨理论尖端距离 390 动程(第 1 牵引点) 119 距心轨理论尖端距离 3955 动程(第 2 牵引点) 59 求解模型得到的计算位移曲线如图2所示,由计算位移曲线确定的长心轨/翼轨间顶铁(a/b)尺寸偏差如表2所示[21],其最大偏差为 −2 mm,小于允许增加的顶铁调整片厚度(3 mm).
表 2 长心轨/翼轨间顶铁尺寸偏差Table 2. Dimensional deviation for distance blocks between long point rail and wing railmm 序号 a b 修正值 计算值 偏差 修正值 计算值 偏差 8# 95 94 1 97 97 0 10# 124 124 0 127 127 0 12# 113 114 −1 116 116 0 14# 152 153 −1 155 155 0 16# 159 161 −2 159 161 −2 19# 159 160 −1 159 159 0 21# 157 157 0 157 156 1 23# 154 154 0 154 155 −1 辙叉最小轮缘槽宽度的计算值为90.7 mm,实测5组辙叉最小轮缘槽宽度的平均值为90.9 mm(见表3).
表 3 18# 可动心轨辙叉最小轮缘槽宽度Table 3. Minimum flangeway width for No. 18 movable point frog序号 1# 2# 3# 4# 5# 平均 检测值/mm 91.0 90.6 90.9 90.6 91.3 90.9 顶铁、最小轮缘槽宽度的计算值与修正值(或实测值)之间偏差较小,验证了本文提出的心轨转换位移曲线数值计算方法的合理性.
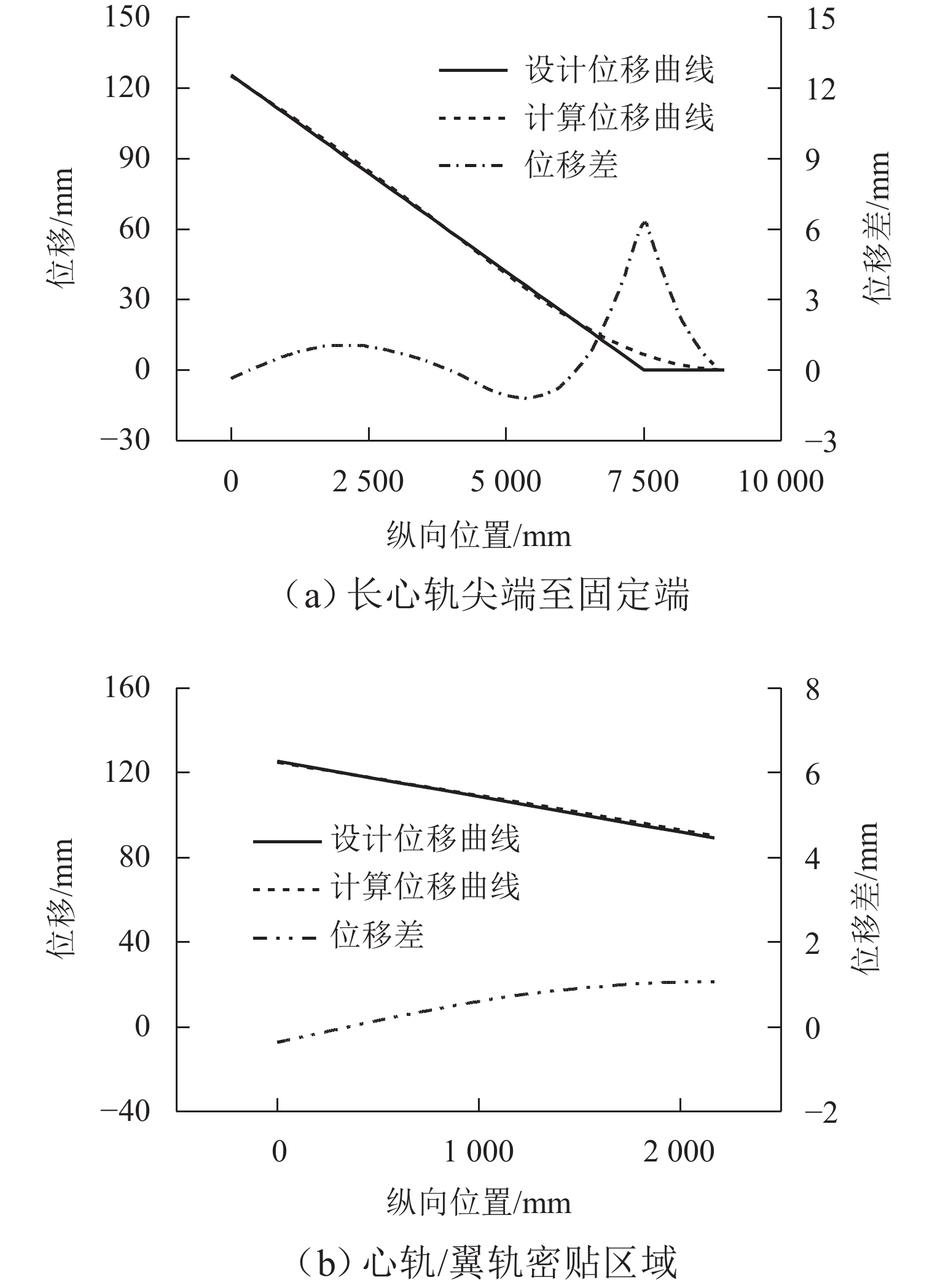
进一步研究计算位移曲线和设计位移曲线偏差,如图3所示. 由图中数据可见,长心轨可动区域(从长心轨尖端至固定端)位移最大偏差为6.64 mm,位于弹性可弯中心,计算位移大于设计位移. 心轨/翼轨密贴段位移最大偏差为1.06 mm,位于密贴段终点,计算位移大于设计位移;心轨尖端位移偏差为0.36 mm,计算位移小于设计位移.
根据计算结果,心轨设计位移曲线和计算位移曲线之间存在偏差,应对转换位移曲线设计方法进行优化. 此外,相较于可动心轨辙叉区最小轮缘槽宽度限值73 mm(辙叉区轨距允许正公差(8 mm)[5-6]和标准轨距的最小轮缘槽宽度限值(65 mm)[7]之和),既有18# 辙叉最小轮缘槽宽度裕量偏大,应适当减小以降低牵引点转换力,使辙叉结构更为合理.
2. 心轨转换位移曲线优化设计方法
基于所建立的心轨转换模型和上文中的计算结果,对18号可动心轨辙叉心轨转换位移曲线进行优化设计.
首先,由心轨转换位移曲线确定的辙叉最小轮缘槽宽度必须满足要求,根据上文中给出的最小轮缘槽宽度限值(73 mm),考虑3 mm的安全裕量,最小轮缘槽宽度目标值按76 mm设置较为合理.
其次,在表1所列出的辙叉关键结构参数中,第2牵引点动程对最小轮缘槽宽度的影响最为显著,二者为正相关关系. 采用减小第2牵引点动程的方法改变心轨转换位移曲线,使最小轮缘槽宽度计算值减小到目标值(最小轮缘槽宽度取值位置为心轨转换位移曲线确定的长心轨(翼)轨密贴段终点). 如前文所述,改变心轨转换位移曲线后,心轨转换模型关键结构参数随之改变,而在第2牵引点动程取值相同的条件下,模型关键结构参数的改变反过来会影响心轨转换位移曲线. 为此,采用逐次逼近的方法进行求解,具体方法为减小所建立心轨转换模型的第2牵引点动程,使最小轮缘槽宽度计算值与目标值偏差≤0.2 mm,采用计算位移曲线确定的关键结构参数修正计算模型. 在第2牵引点动程取值不变的条件下进行校核计算,当最小轮缘槽宽度满足要求(计算值和校核值偏差≤0.2 mm)时终止计算,不满足时则重复上述调整、修正和校核计算过程,直至满足要求,整体流程如图4所示,初始设计计算工况如表4所示.
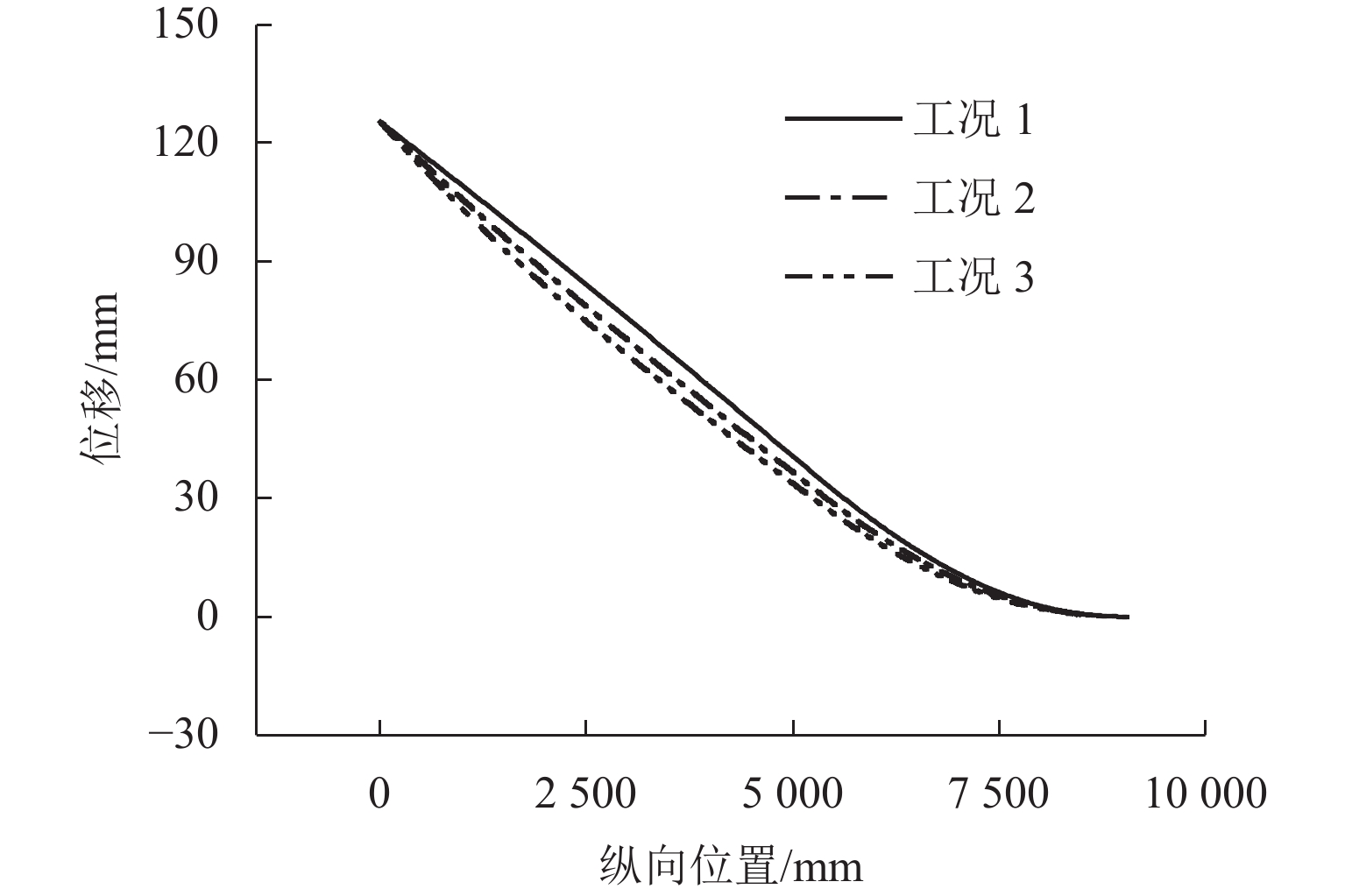
表 4 数值计算工况Table 4. Numerical calculation conditionsmm 工况 第 2 牵引点动程 最小轮缘槽宽度 1(初始设计) 59.0 90.7 2 54.0 81.9 3 50.5 76.1 不同工况的计算位移曲线模型见图5. 工况2最小轮缘槽宽度(81.9 mm)安全裕量仍然过大,工况3最小轮缘槽宽度(76.1 mm)比目标值仅大0.1 mm,因此,选择工况3进行下一步计算. 基于工况3计算位移曲线得出的关键结构参数(见表5),建立修正模型1,并补充计算工况4第2牵引点动程(修正模型1)为50.5 mm,重新进行计算. 工况4计算位移曲线如图6,最小轮缘槽宽度为75.6 mm,与工况3相比,其最小轮缘槽宽度计算结果偏差为0.5 mm,且小于限值要求.
表 5 初始模型和修正模型1关键结构参数Table 5. Key structure parameters for initial model and refined model 1mm 关键参数 长心轨轨头
加工长短心轨轨头
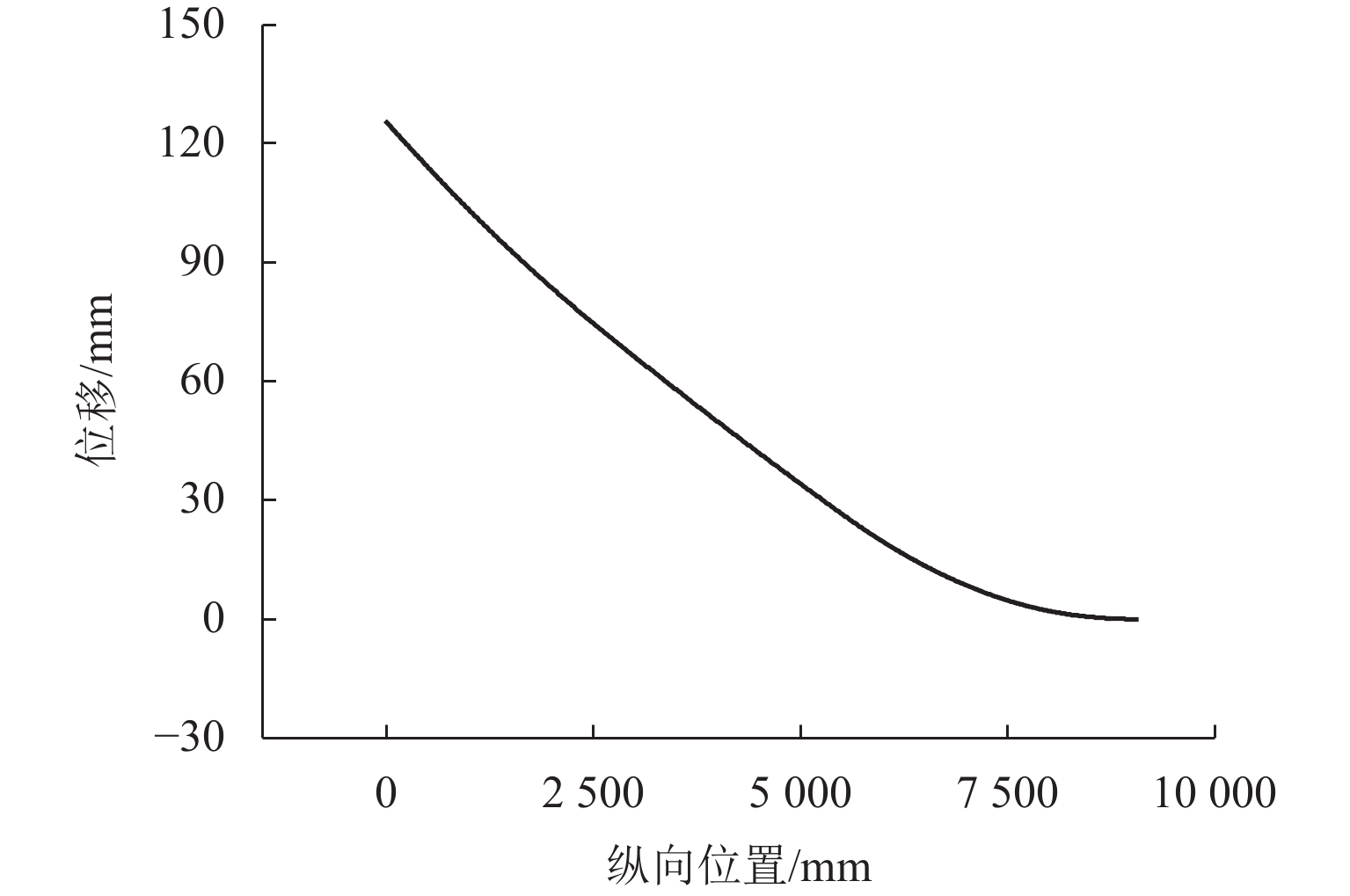
加工长短心轨长 初始模型 2169 2075 8215 修正模型 1 2425 2056 7959 将修正模型1的第2牵引点动程调整为50.7 mm,补充工况5进行计算. 工况5计算位移曲线如图7所示,最小轮缘槽宽度为76.0 mm,最小轮缘槽宽度满足要求. 基于工况5计算位移曲线得出的关键结构参数(见表6),建立修正模型2,并补充计算工况6(第2牵引点动程为50.7 mm),重新进行计算.
表 6 修正模型1和修正模型2关键结构参数Table 6. Key structure parameters for refined model 1 and refined model 2mm 关键参数 长心轨轨头
加工长短心轨轨头
加工长短心轨长 修正模型 1 2425 2056 7959 修正模型 2 2427 2020 7957 工况6计算位移曲线如图8所示,其最小轮缘槽宽度与工况5一致,为76.0 mm.
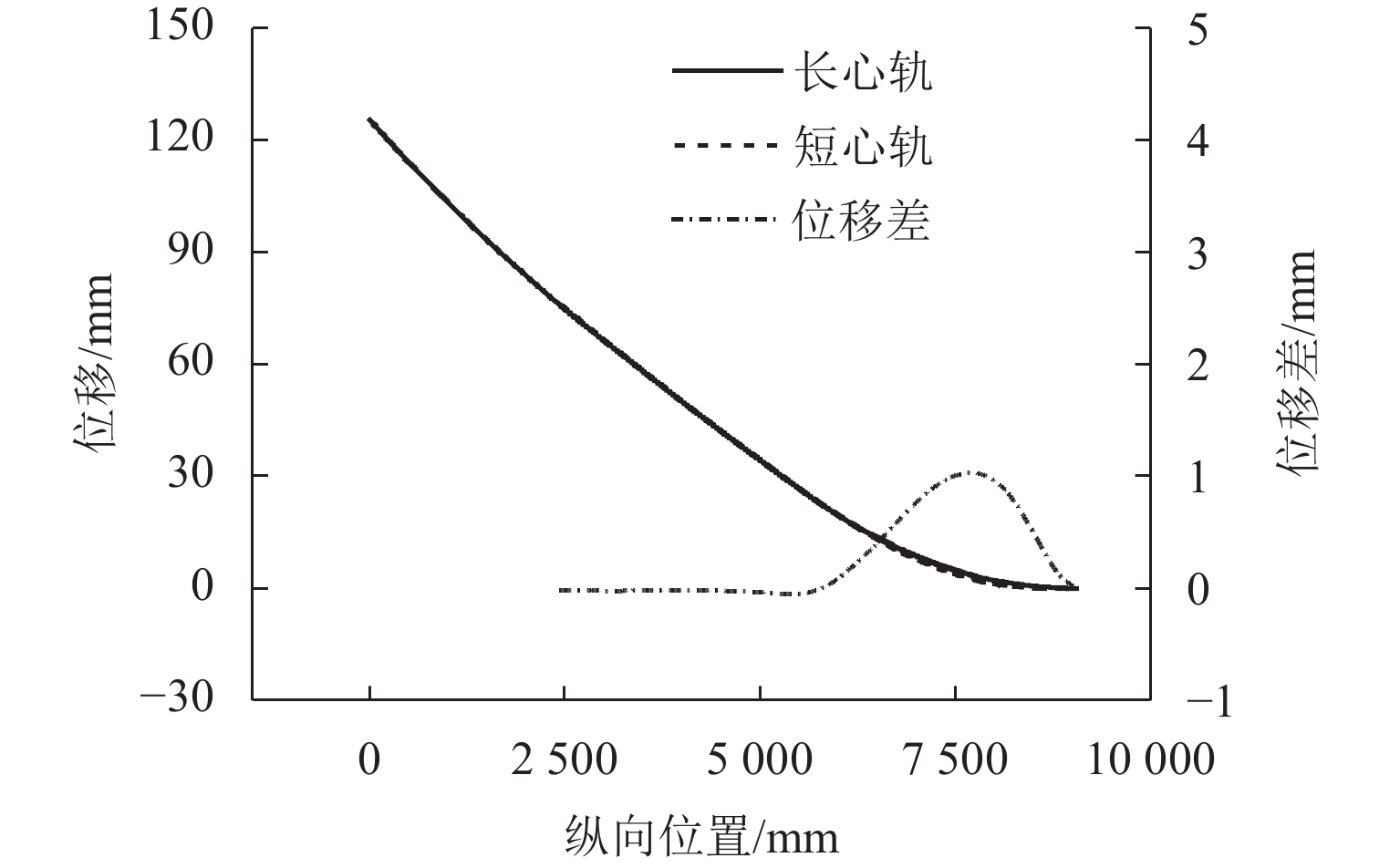
采用工况6计算位移曲线得出的关键结构参数与修正模型2关键结构参数值一致,见表7. 至此,迭代仿真结束,工况6牵引动程及计算位移曲线为最终优化结果. 另外,工况6长心轨和短心轨计算位移差异见图9,可以看出,自短心轨尖端起向后3.4 m范围内,长、短心轨位移基本一致;距尖端3.4 m到距尖端5.4 m范围内,长心轨位移逐渐大于短心轨,位移差最大值为1.04 mm,出现在距短心轨尖端5.4 m位置;自距短心轨尖端5.4 m至长心轨固定端范围内位移差异逐渐减小为0.
表 7 关键结构参数比较Table 7. Key structure parameter comparisonmm 关键参数 修正模型 2 工况 6 长心轨轨头加工长 2427 2427 短心轨轨头加工长 2020 2020 短心轨长 7957 7957 综上所述,采用最小轮缘槽宽度作为确定心轨转换位移曲线的关键参数,基于所建立的心轨转换模型,采用逐次逼近法进行求解,通过设置6个计算工况,更新2次计算模型后,工况6趋于收敛,因此,选择工况6计算的位移曲线作为18号可动心轨辙叉心轨转换位移曲线的优化设计方案.
3. 可动心轨辙叉关键部件设计方法
基于心轨转换位移曲线,开展关键部件结构设计. 相对于侧向,可动心轨辙叉直向通过速度更高(目前直向过岔最高速度为350 km/h[22]),对辙叉直向几何形位公差的要求也更高[3-4],考虑上述因素,可动心轨辙叉关键部件按直向开通设计.
1) 长心轨
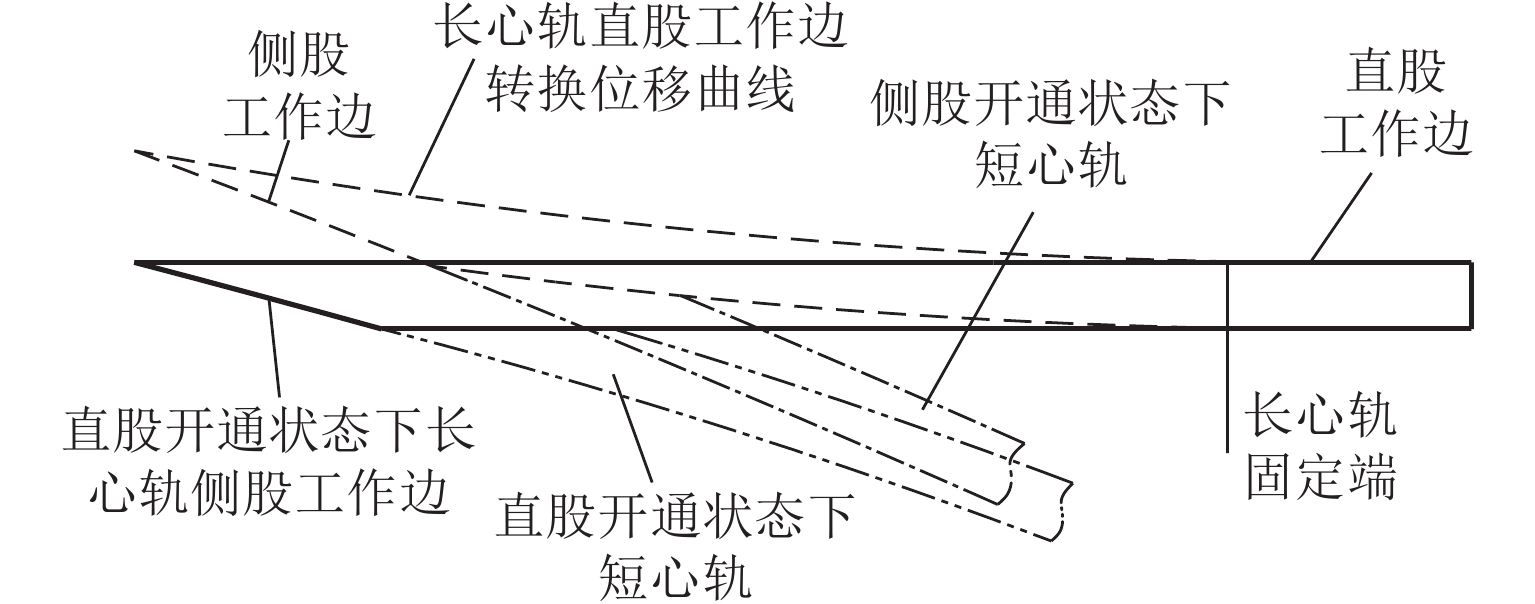
长心轨为可动心轨辙叉关键部件(见图10),与短心轨组装为可动心轨,在转辙机牵引下与不同侧翼轨密贴形成直、侧向连续轨线,确定直股开通状态下长心轨侧股工作边线型是长心轨设计的关键.
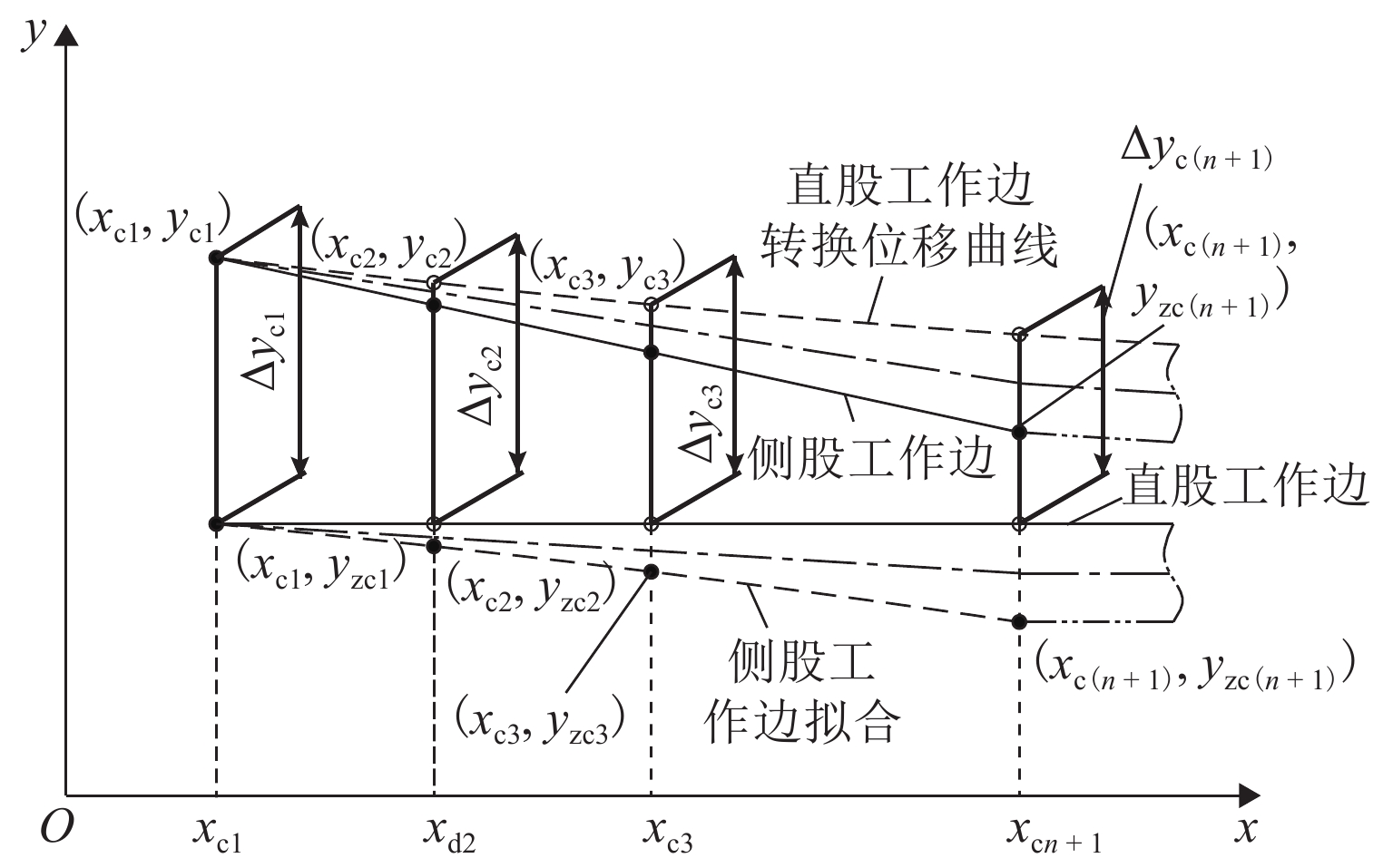
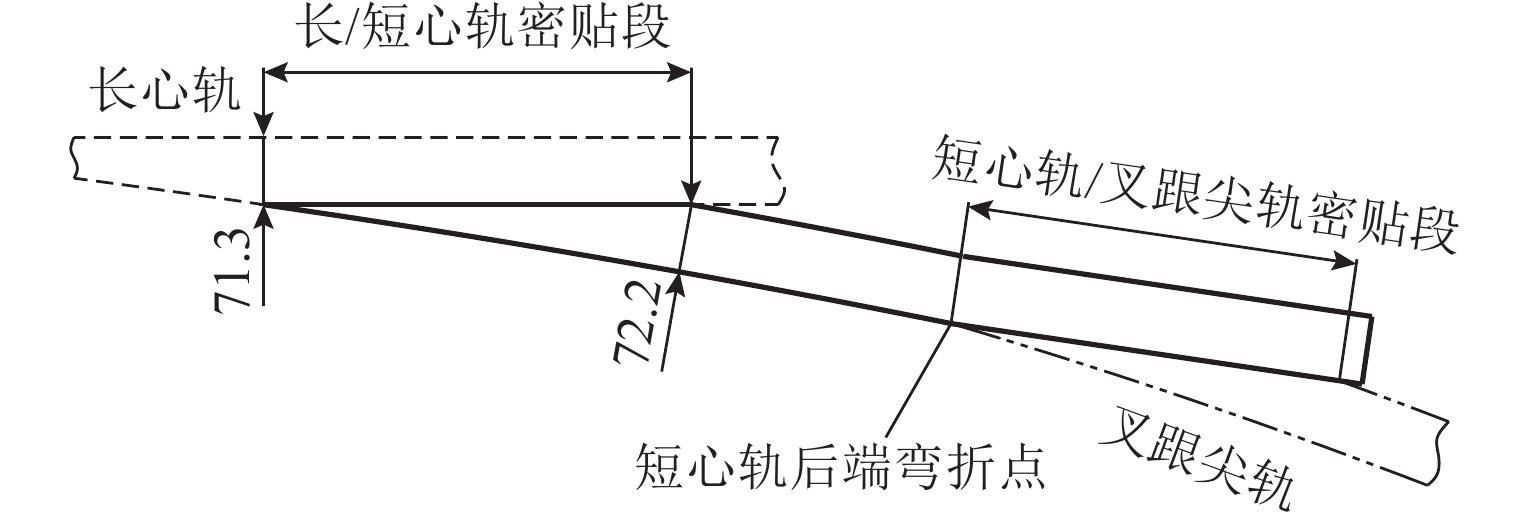
其设计方法为:基于上文计算得到的心轨直股工作边转换位移曲线和侧股工作边,可确定长心轨/翼轨密贴段(心轨理论尖端至71.3 mm断面)长度及密贴段范围内长心轨横向位移量Δyc,将长心轨/翼轨密贴段沿轨道纵向离散为n段,共n + 1个节点,各节点位置坐标分别为xc1、xc2、…、xc(n + 1),对应的横向位移量分别为Δyc1、Δyc2、…、Δyc(n + 1). 采用同样方法,将长心轨/翼轨密贴段范围内的侧股工作边进行离散,可得侧股工作边各节点对应横向坐标yci,其中i = 1,2, …,n + 1. 对各个离散节点Pci,分别依据其侧股工作边坐标yci和转换后的横向位移量Δyci,叠加计算得到直股开通状态下长心轨侧股工作边各节点的横向位置坐标yzci如式(1)所示.
yzci=yci−Δyci,i=1,2,⋯,n+1. (1) 基于直股开通状态下,长心轨非工作边各节点坐标(xci, yzci),采用样条曲线拟合各节点,得到直股开通状态下长心轨侧股工作边线型,见图11.
长心轨其他结构的设计方法可参照既有设计方法进行.
2) 短心轨
短心轨与长心轨通过联结零件组装为整体. 短心轨尖端至72.2 mm的断面与长心轨密贴;后端弯折点至跟端与叉跟尖轨密贴,转换时,后端弯折点至跟端线型不变,如图12所示. 直股开通时,短心轨与长心轨密贴段非工作边为水平线,确定直股开通状态下短心轨尖端至后端弯折点工作边线型是短心轨设计的关键.
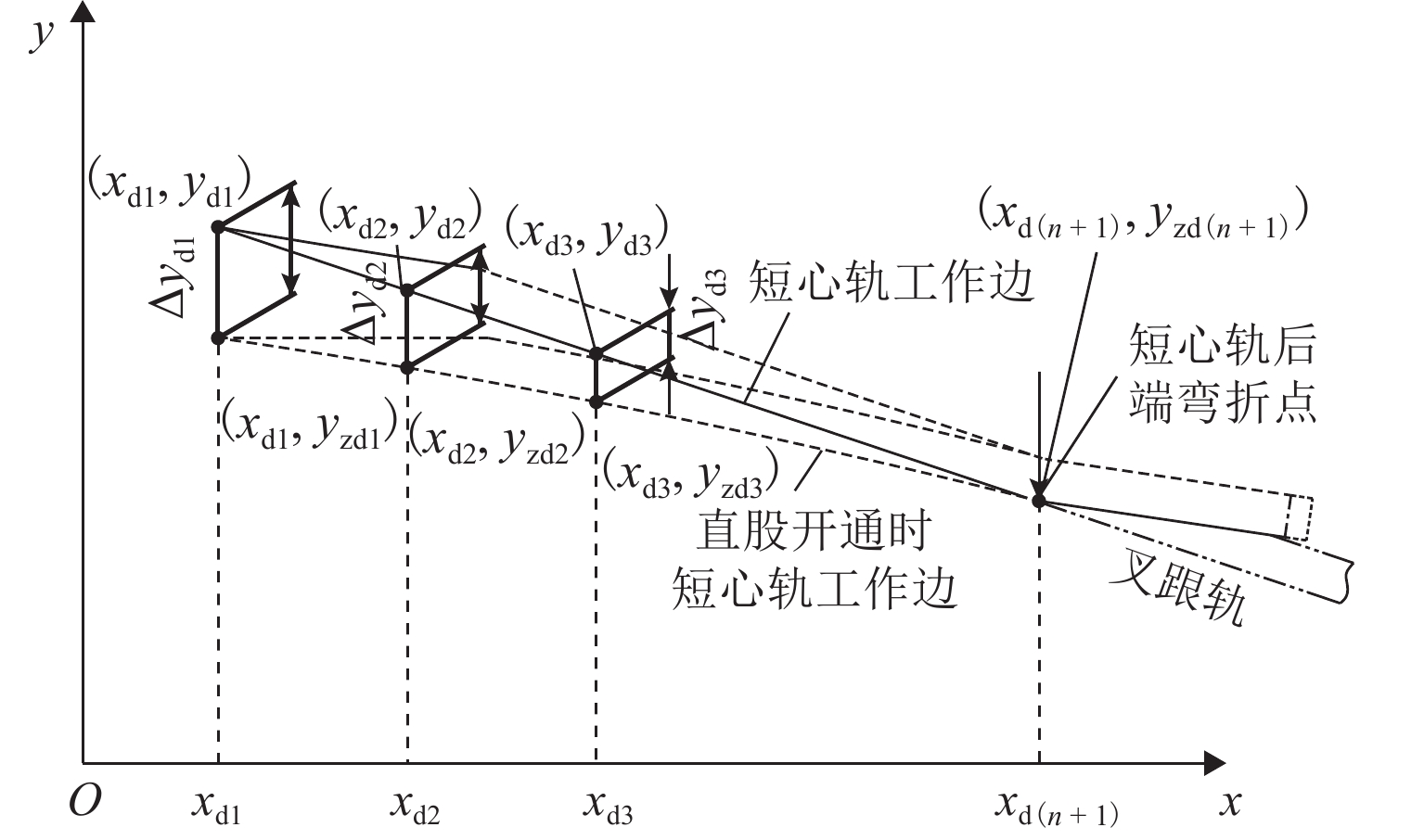
基于上文计算得到的短心轨工作边转换位移曲线,可确定短心轨尖端至短心轨后端弯折点的横向位移Δyd,采用与长心轨相同的离散方法,将短心轨尖端至后端弯折点沿轨道纵向离散为n段,共n + 1个节点,各节点位置坐标分别为xd1、xd2、…、xd(n + 1),对应的横向位移量分别为Δyd1、Δyd2、…、Δyd(n + 1). 将短心轨尖端至后端弯折点对应范围内的侧股工作边进行离散,可得各节点对应的侧股工作边横向坐标yd1、yd2、…、yd(n + 1). 对各离散节点Pdi(i=1,2,…,n + 1),分别依据其侧股工作边坐标ydi和转换后的横向位移量Δydi,叠加计算得到直股开通状态下短心轨尖端至后端弯折点工作边各节点横向位置坐标yzdi如式(2)所示.
yzdi=ydi−Δydi,i=1,2,⋯,n+1. (2) 基于直股开通状态下,短心轨工作边各节点坐标(xdi, yzdi),采用样条曲线拟合各节点,得到直股开通状态下短心轨工作边线型,如图13所示.
短心轨其他结构的设计方法可参照既有设计方法进行.
3) 翼轨
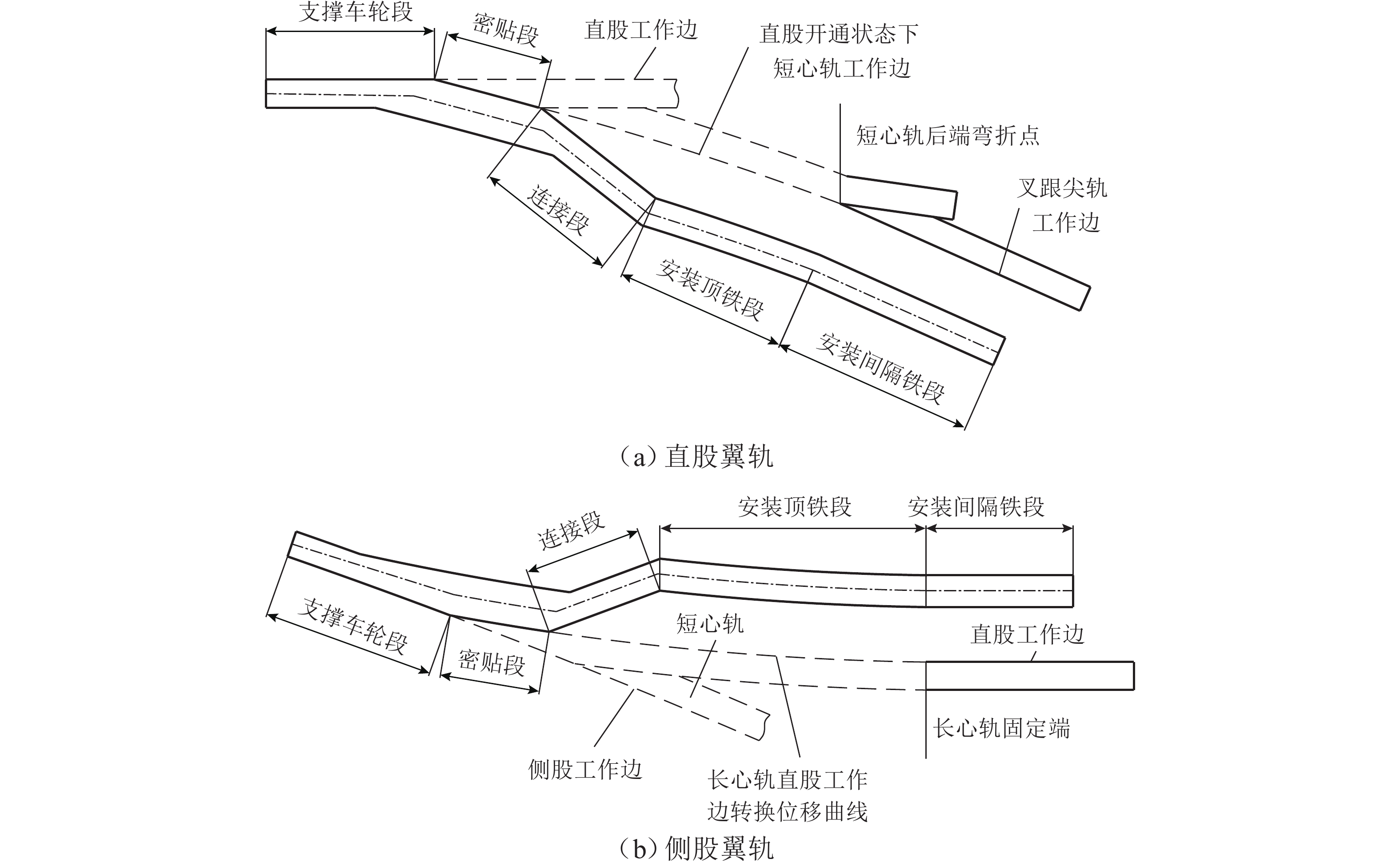
可动心轨辙叉翼轨分为直股翼轨和侧股翼轨,辙叉开通直股时与心轨密贴的翼轨为直股翼轨,开通侧股时与心轨密贴的翼轨为侧股翼轨. 按照不同的功能,将翼轨工作边可分为支撑车轮段、密贴段、连接段、安装顶铁段和安装间隔铁段. 确定各段翼轨工作边为翼轨设计的关键,见图14.
直股翼轨工作边设计方法为:支撑车轮段工作边线型采用辙叉直股工作边;为与直股开通状态下心轨密贴,密贴段工作边线型采用上文所述直股开通状态下长心轨非工作边线型;为减少顶铁规格,安装顶铁段的工作边线型采用上文所述直股开通状态下短心轨工作边线型偏移得到;为简化间隔铁结构,安装间隔铁段的线型采用叉跟尖轨工作边偏移得到(叉跟尖轨工作边由道岔总体线型确定).
侧股翼轨工作边设计方法为:支撑车轮段工作边线型为辙叉侧股工作边线型;为与处于侧股开通状态下心轨密贴,密贴段工作边线型采用上文所述心轨直股工作边计算位移曲线;为减少顶铁规格,翼轨安装顶铁段的工作边线型采用直股工作边计算位移曲线偏移得到;为简化间隔铁结构,翼轨安装间隔铁段的线型采用直股工作边偏移得到.
工作边确定后,应根据具体结构调整连接段工作边,合理布置弯折点位置,使各弯折点弯折角度均匀便于制造.
4) 长/短心轨间联结零部件
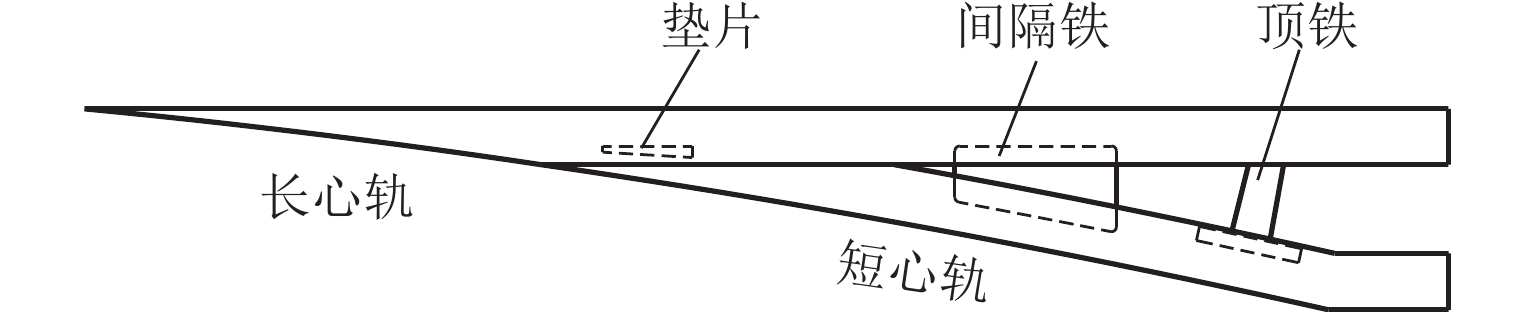
心轨组件由长心轨、短心轨、联结零部件(间隔铁、顶铁等)采用高强螺栓联结为整体,如图15所示.
由于长、短心轨转换时存在位移差异,将长、短心轨联结为整体的间隔铁,应设置在长心轨、短心轨位移差异较小的区域,避免扰动辙叉线型;同理,设置于心轨组件后端的顶铁,受长、短心轨转换位移差的影响,直股开通时的尺寸小于侧股开通时的尺寸,为避免影响直向工作边直线度,其尺寸应按直股开通时设计,当转换至侧股时,顶铁会与长心轨轨腰存在间隙,间隙的大小取决于顶铁位置处长、短心轨位移差.
4. 结束语
本文以18号可动心轨辙叉为研究对象,分析既有心轨转换位移曲线设计方法的不足,基于有限元方法建立心轨转换模型,对心轨转换位移曲线进行了仿真计算,结果表明:心轨计算转换位移与设计转换位移最大偏差达到6.64 mm,位于弹性可弯中心;密贴段最大位移偏差为1.06 mm,位于密贴段终点;心轨尖端位移偏差为0.36 mm,可对心轨转换位移曲线设计方法进行优化,以减小心轨/翼轨密贴间隙. 此外,最小轮缘槽宽度计算值(90.7 mm)和实测平均值(90.9 mm)差异较小,但与73 mm的限值相比裕量较大,可适当减小最小轮缘槽宽度以降低牵引点转换力,使辙叉结构更为合理.
为此,基于所建立的心轨转换模型,提出了以最小轮缘槽宽度为关键指标,采用逐次逼近求解的方式对心轨转换位移曲线进行优化设计的方法,并给出最小轮缘槽宽度目标值. 针对用量最大的18号可动心轨辙叉,通过计算提出了牵引点动程及心轨转换曲线优化方案. 在此基础上,根据辙叉直、侧向不同的形位公差要求,提出了按直向开通状态进行结构设计的原则和关键部件设计方法.
下一步工作,拟基于计算位移曲线分布,确定长、短心轨之间间隔铁和顶铁设置位置和尺寸,在计算模型中增加顶铁和间隔铁后进行仿真计算,探明间隔铁和顶铁对转换位移曲线的影响规律;另外,在本文研究工作基础上,还可开展心轨磨耗后对转换位移曲线的影响;拟采用本文提出的方法进行下一代可动心轨辙叉结构设计,试制新辙叉并开展室内试验,对数值计算和设计方法进行进一步的验证.
致谢:中国铁道科学研究院集团有限公司科研项目(2022YJ177).
-
表 1 18号可动心轨辙叉关键结构参数
Table 1. Key structure parameters for No. 18 movable point frog
结构参数 取值/mm 长心轨轨头加工长度 2169 短心轨轨头加工长度 2075 短心轨长度 8215 长心轨可动段长度 9055 弹性可弯中心距心轨理论尖端距离 7505 咽喉宽度 125 距心轨理论尖端距离 390 动程(第 1 牵引点) 119 距心轨理论尖端距离 3955 动程(第 2 牵引点) 59 表 2 长心轨/翼轨间顶铁尺寸偏差
Table 2. Dimensional deviation for distance blocks between long point rail and wing rail
mm 序号 a b 修正值 计算值 偏差 修正值 计算值 偏差 8# 95 94 1 97 97 0 10# 124 124 0 127 127 0 12# 113 114 −1 116 116 0 14# 152 153 −1 155 155 0 16# 159 161 −2 159 161 −2 19# 159 160 −1 159 159 0 21# 157 157 0 157 156 1 23# 154 154 0 154 155 −1 表 3 18# 可动心轨辙叉最小轮缘槽宽度
Table 3. Minimum flangeway width for No. 18 movable point frog
序号 1# 2# 3# 4# 5# 平均 检测值/mm 91.0 90.6 90.9 90.6 91.3 90.9 表 4 数值计算工况
Table 4. Numerical calculation conditions
mm 工况 第 2 牵引点动程 最小轮缘槽宽度 1(初始设计) 59.0 90.7 2 54.0 81.9 3 50.5 76.1 表 5 初始模型和修正模型1关键结构参数
Table 5. Key structure parameters for initial model and refined model 1
mm 关键参数 长心轨轨头
加工长短心轨轨头
加工长短心轨长 初始模型 2169 2075 8215 修正模型 1 2425 2056 7959 表 6 修正模型1和修正模型2关键结构参数
Table 6. Key structure parameters for refined model 1 and refined model 2
mm 关键参数 长心轨轨头
加工长短心轨轨头
加工长短心轨长 修正模型 1 2425 2056 7959 修正模型 2 2427 2020 7957 表 7 关键结构参数比较
Table 7. Key structure parameter comparison
mm 关键参数 修正模型 2 工况 6 长心轨轨头加工长 2427 2427 短心轨轨头加工长 2020 2020 短心轨长 7957 7957 -
[1] 徐井芒,郑兆光,赖军,等. 轨道参数对高速道岔轮轨接触行为的影响[J]. 西南交通大学学报,2022,57(5): 990-999. doi: 10.3969/j.issn.0258-2724.20210449XU Jingmang, ZHENG Zhaoguang, LAI Jun, et al. Influence of track parameters on wheel/rail contact behavior of high-speed turnout[J]. Journal of Southwest Jiaotong University, 2022, 57(5): 990-999. doi: 10.3969/j.issn.0258-2724.20210449 [2] 郝瀛. 铁道工程[M]. 北京:中国铁道出版社,2000:98. [3] 国家铁路局. 高速铁路道岔制造技术条件. 第1部分:制造与组装:TB/T 3307.1—2020[S]. 北京:中国铁道出版社,2020. [4] 国家铁路局. 标准轨距铁路道岔:TB/T 412—2020[S]. 北京:中国铁道出版社,2020. [5] 国家铁路局. 高速铁路无砟轨道线路维修规则(试行): TG/GW 115—2012[Z]. 北京:中国铁道出版社,2012. [6] 国家铁路局. 高速铁路有砟轨道线路维修规则(试行): TG/GW 116—2013[Z]. 北京:中国铁道出版社,2013. [7] 国家铁路局. 普速铁路线路修理规则:TG/GW 102—2019[Z]. 北京: 中国铁道出版社,2020. [8] 王树国,王璞,赵振华,等. 高铁正线道岔专项评估整治调研报告[R]. 北京:中国铁道科学研究院集团有限公司,2022. [9] 蔡小培,李成辉. 高速道岔心轨扳动力和不足位移控制研究[J]. 铁道学报,2008,30(2): 48-51. doi: 10.3321/j.issn:1001-8360.2008.02.010CAI Xiaopei, LI Chenghui. Study on controlling the swiching force and scant displacement of the point rail of the high speed turnout[J]. Journal of the China Railway Society, 2008, 30(2): 48-51. doi: 10.3321/j.issn:1001-8360.2008.02.010 [10] 曾瑞东,王树国,王璞,等. 跟端轨底刨切对尖轨转换影响的有限元分析[J]. 铁道建筑,2019,59(2): 140-142,146. doi: 10.3969/j.issn.1003-1995.2019.02.33ZENG Ruidong, WANG Shuguo, WANG Pu, et al. FEM simulation analysis about influence of rail bottom slicing on switch rail transformation[J]. Railway Engineering, 2019, 59(2): 140-142,146. doi: 10.3969/j.issn.1003-1995.2019.02.33 [11] 马晓川,王平. 小号码道岔扳动力随密贴段刚度变化规律研究[J]. 铁道标准设计,2014,58(5): 12-14,20.MA Xiaochuan, WANG Ping. Study on change rule of switching force of small-sized turnout with the change of closure section’s stiffness[J]. Railway Standard Design, 2014, 58(5): 12-14,20. [12] 司道林,赵振华,王树国,等. 新型50 kg•m−1钢轨9号道岔尖轨转换特性研究[J]. 中国铁道科学,2021,42(4): 27-33. doi: 10.3969/j.issn.1001-4632.2021.04.04SI Daolin, ZHAO Zhenhua, WANG Shuguo, et al. Study on switching characteristics of switch rail for No. 9 turnout of new 50 kg•m−1 rail[J]. China Railway Science, 2021, 42(4): 27-33. doi: 10.3969/j.issn.1001-4632.2021.04.04 [13] Čedomir I, Mirjana Tomičić-Torlaković, Gordan Radivojević. Modelling the swing nose frog of turnout[J]. Architecture and Civil Engineer Vol.1, 1997, 4: 533-539. [14] 井国庆. 18号可动心轨道岔心轨不足位移仿真分析[D]. 成都:西南交通大学,2006. [15] 王树国. 我国铁路道岔现状与发展[J]. 铁道建筑,2015,55(10): 42-46. doi: 10.3969/j.issn.1003-1995.2015.10.08WANG Shuguo. Current status and future development trend of railway turnouts in China[J]. Railway Engineering, 2015, 55(10): 42-46. doi: 10.3969/j.issn.1003-1995.2015.10.08 [16] 沈长耀,王明治,许有全,等. 提速道岔平面及结构设计[J]. 铁道标准设计,1997(3): 7-13. [17] 葛晶,杨东升,等. 全路铁路道岔型号梳理[R]. 北京:中国铁道科学研究院集团有限公司,2022. [18] 王璞,杨东升,赵振华,等. 60 kg/m钢轨12号单开道岔转换优化数值分析与试验研究[J]. 工程力学,2023,40(7):249-256.WANG Pu, YANG Dongsheng, ZHAO Zhenhua , et al. Numerical analysis and experimental research on switching optimization of the No. 12 single turnout with 60 kg/m rails[J]. Engineering Mechanics, 2023, 40(7):249-256. [19] 蔡小培,李成辉,王平. 滑床板摩擦力对尖轨不足位移的影响[J]. 中国铁道科学,2007,28(1): 8-12. doi: 10.3321/j.issn:1001-4632.2007.01.002CAI Xiaopei, LI Chenghui, WANG Ping. Effect on the scant displacement of switch rail induced by friction of slide baseplate[J]. China Railway Science, 2007, 28(1): 8-12. doi: 10.3321/j.issn:1001-4632.2007.01.002 [20] 于浩,王平,温静,等. 可动心轨转换锁闭力仿真研究[J]. 铁道科学与工程学报,2020,17(3): 533-539.YU Hao, WANG Ping, WEN Jing, et al. Simulation and analysis of the movable rail conversion and locking force[J]. Journal of Railway Science and Engineering, 2020, 17(3): 533-539. [21] 赵振华. 自主研发高速道岔制造标准化研究报告[R]. 北京:中国铁道科学研究院集团有限公司,2021. [22] 王平,刘学毅,陈嵘. 我国高速铁路道岔技术的研究进展[J]. 高速铁路技术,2010,1(2): 6-13. doi: 10.3969/j.issn.1674-8247.2010.02.002WANG Ping, LIU Xueyi, CHEN Rong. Progress of turnout technology for China’s high speed railway[J]. High Speed Railway Technology, 2010, 1(2): 6-13. doi: 10.3969/j.issn.1674-8247.2010.02.002 -





 下载:
下载:















 下载:
下载: