Research on Time Lag Control of Levitation System of Two-Degree-of-Freedom Magnetic Levitation Train
-
摘要:
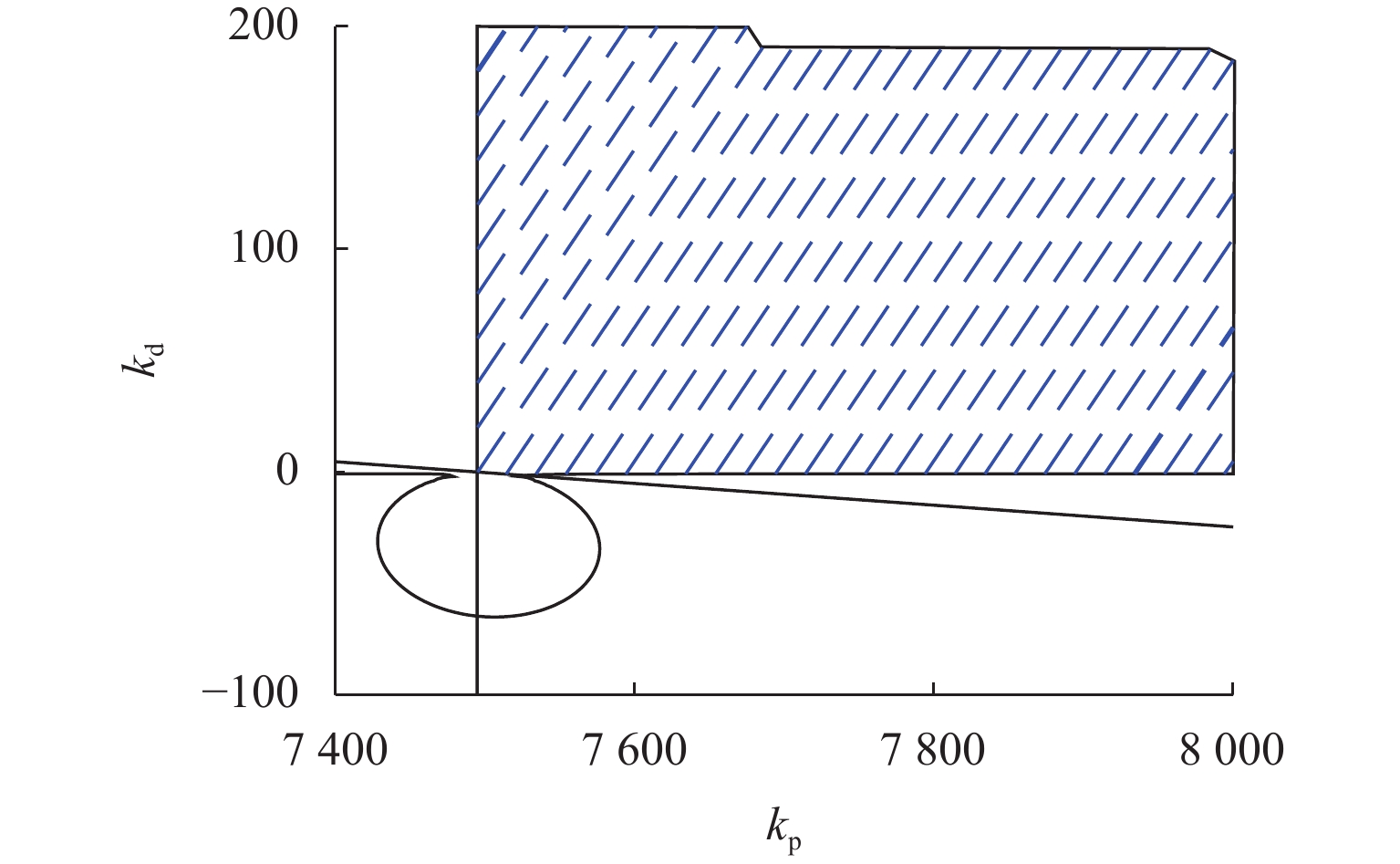
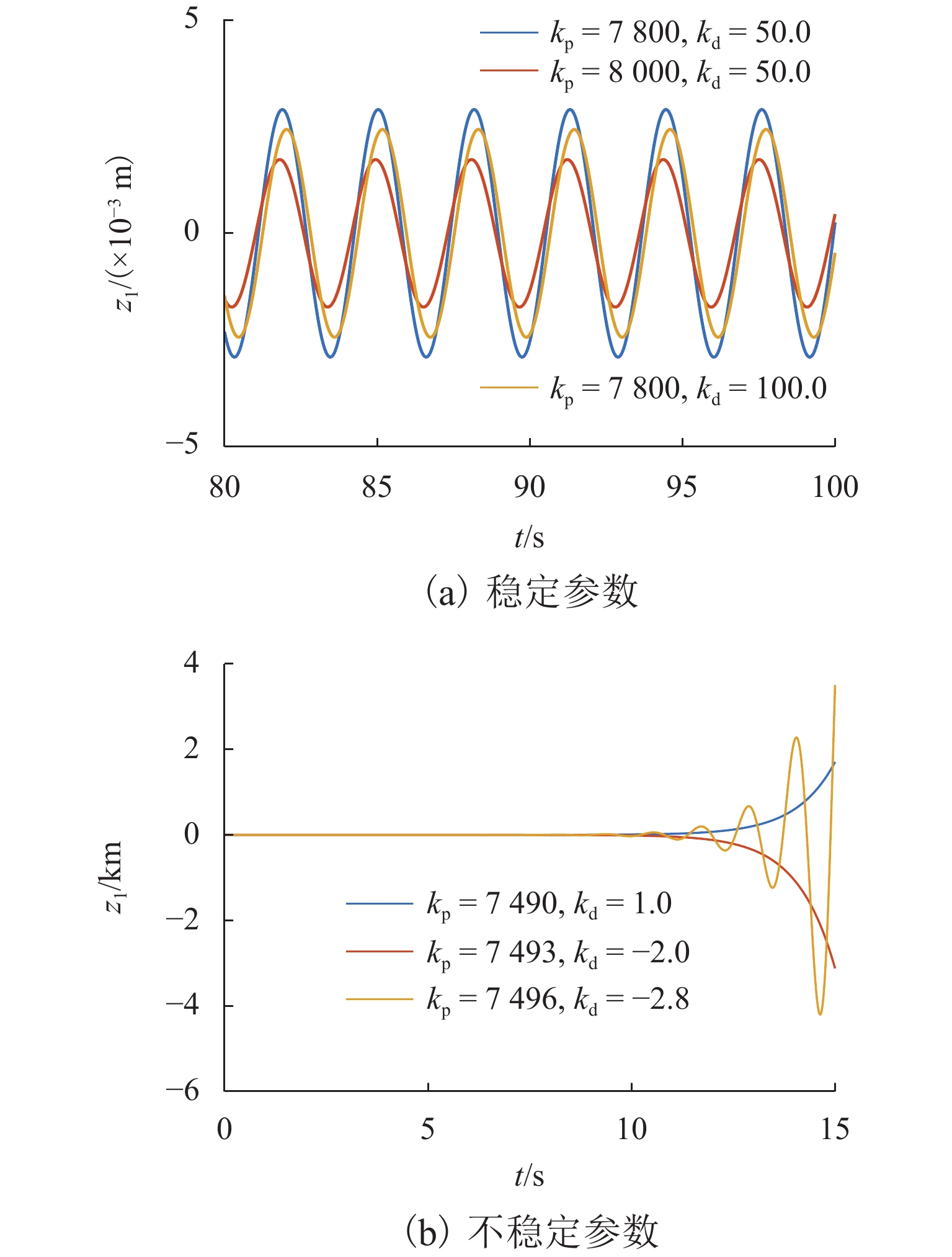
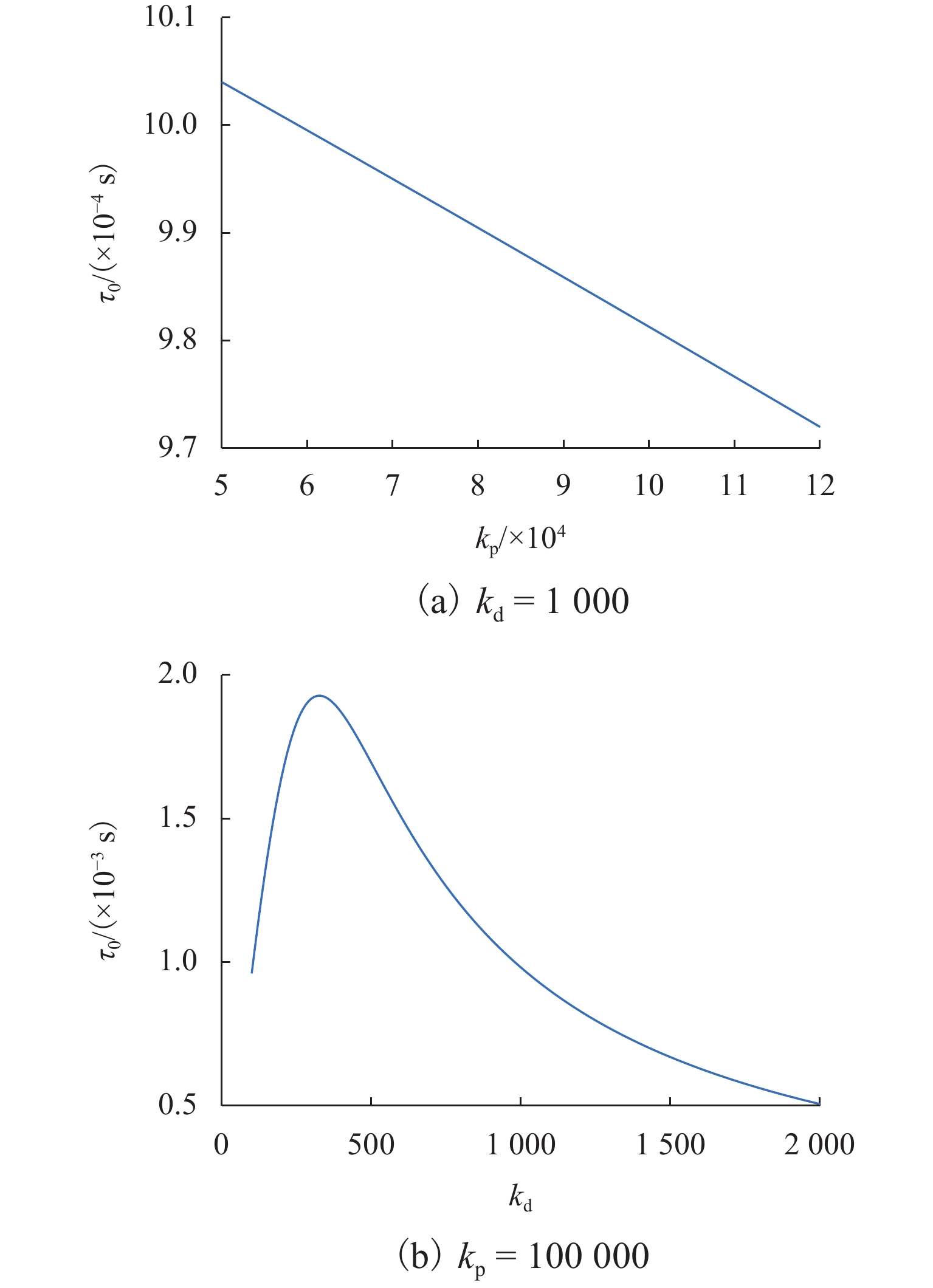
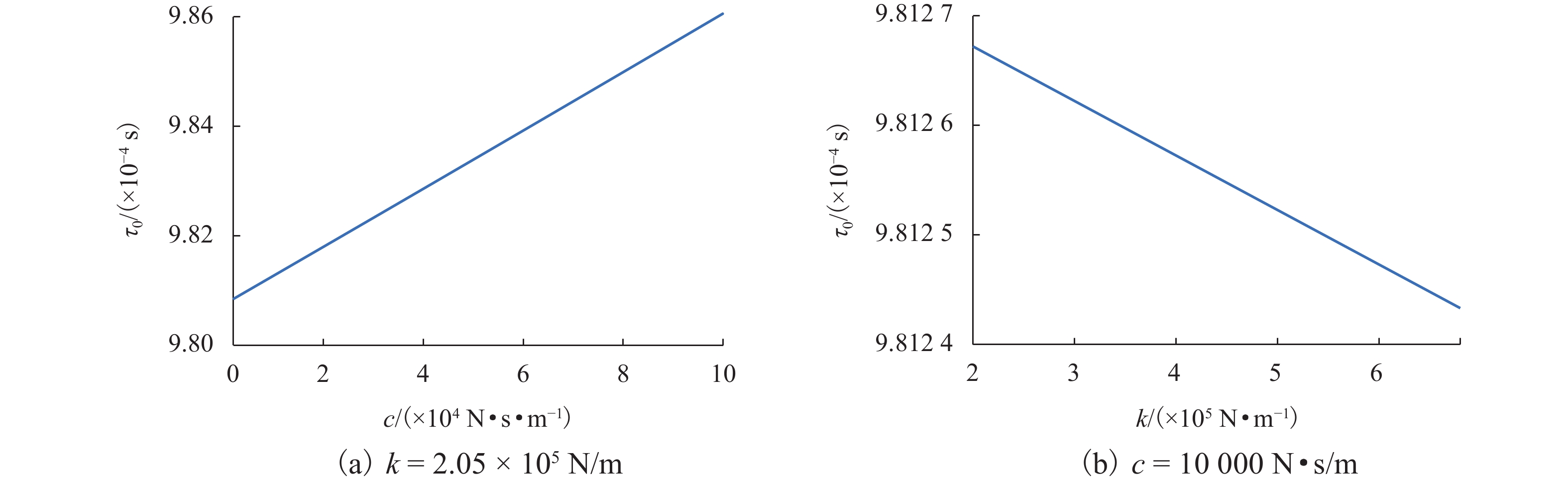
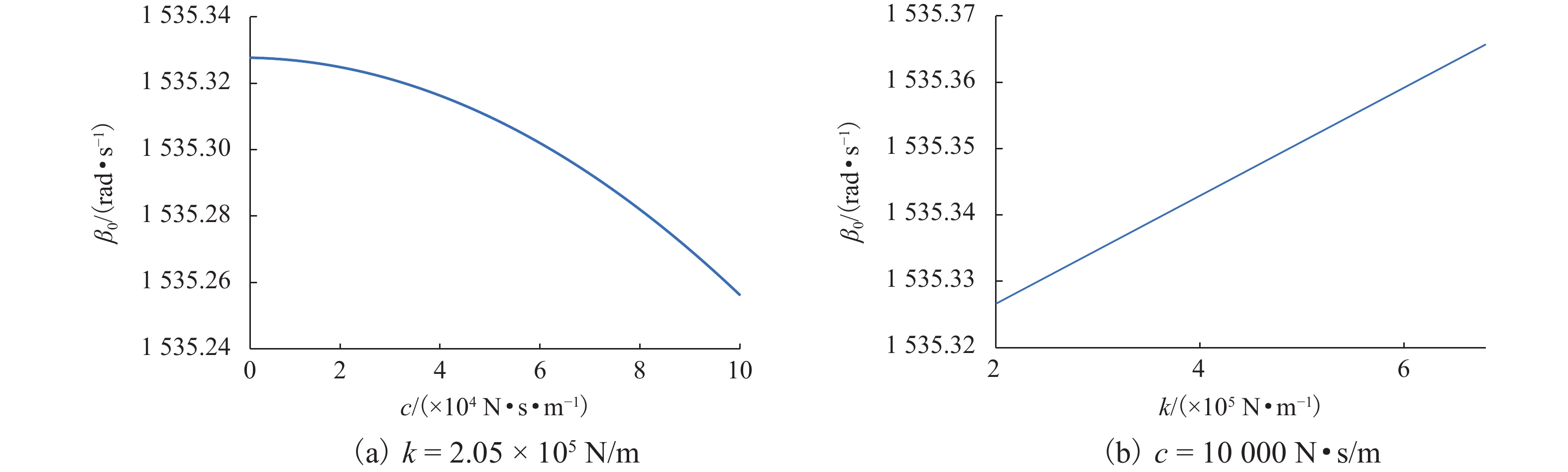
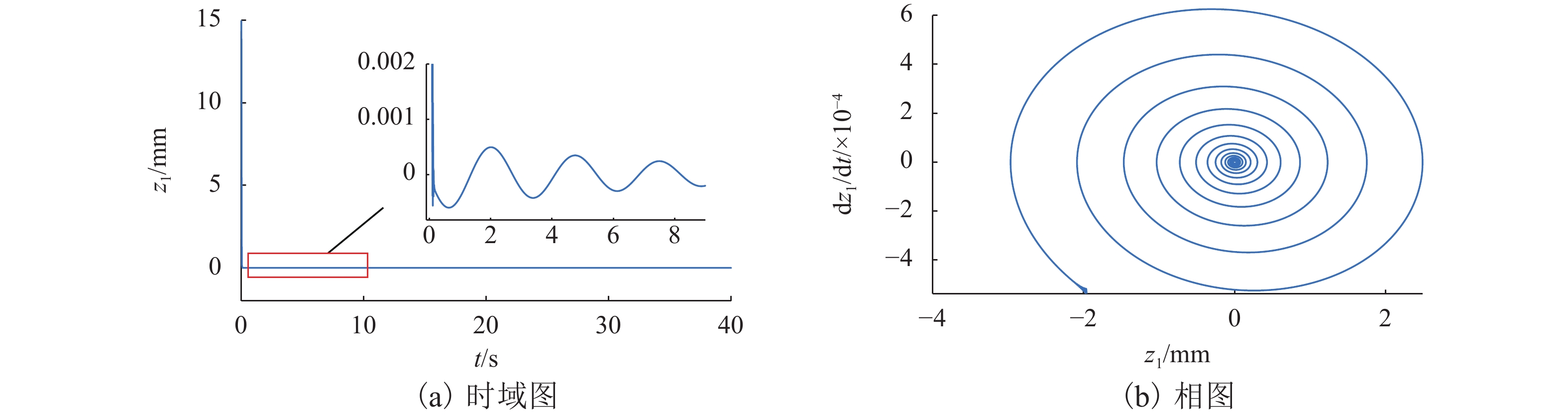
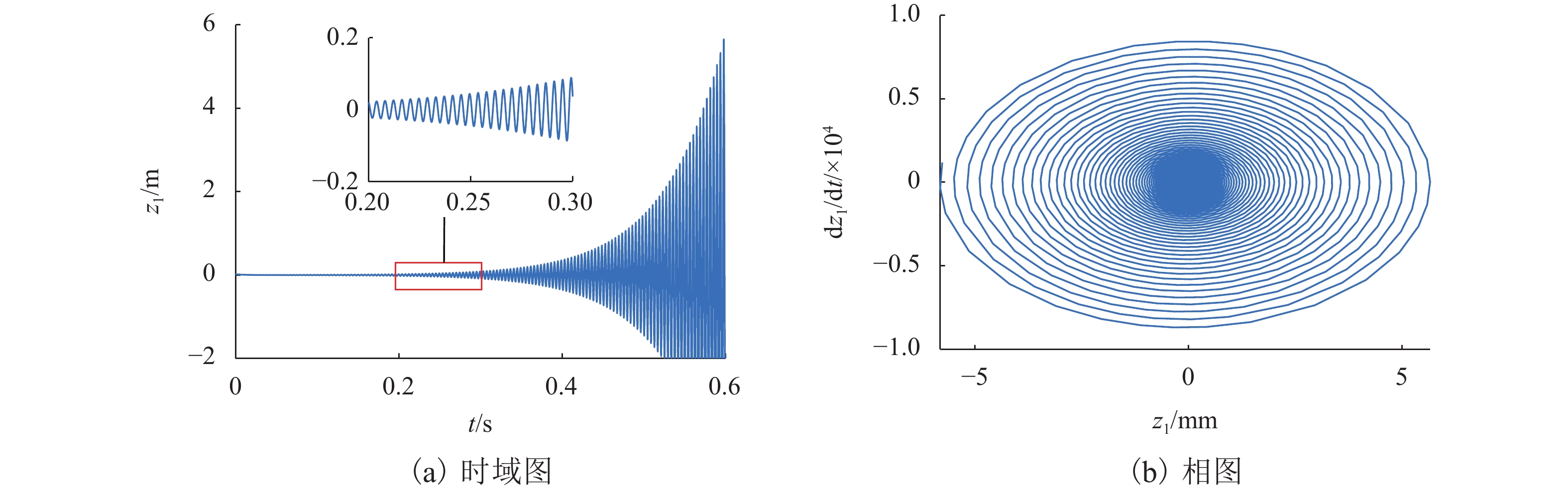
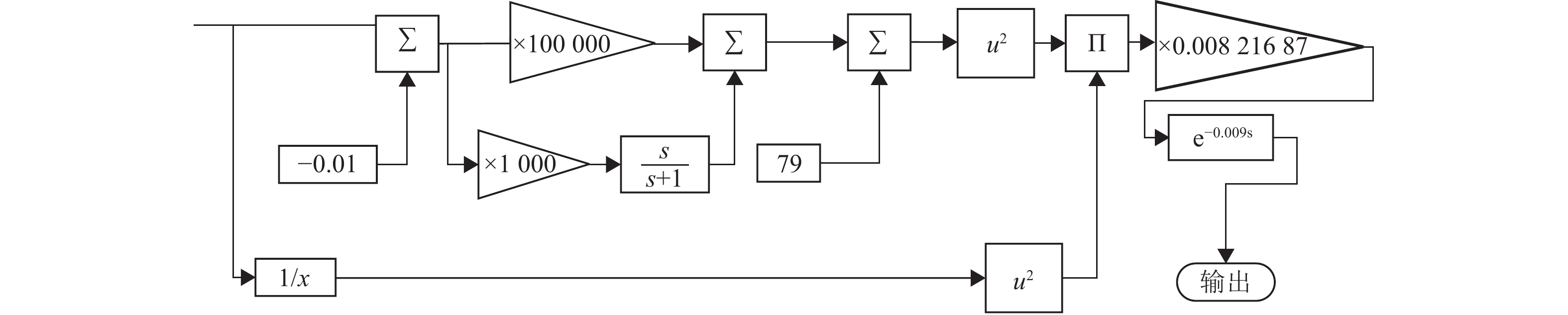
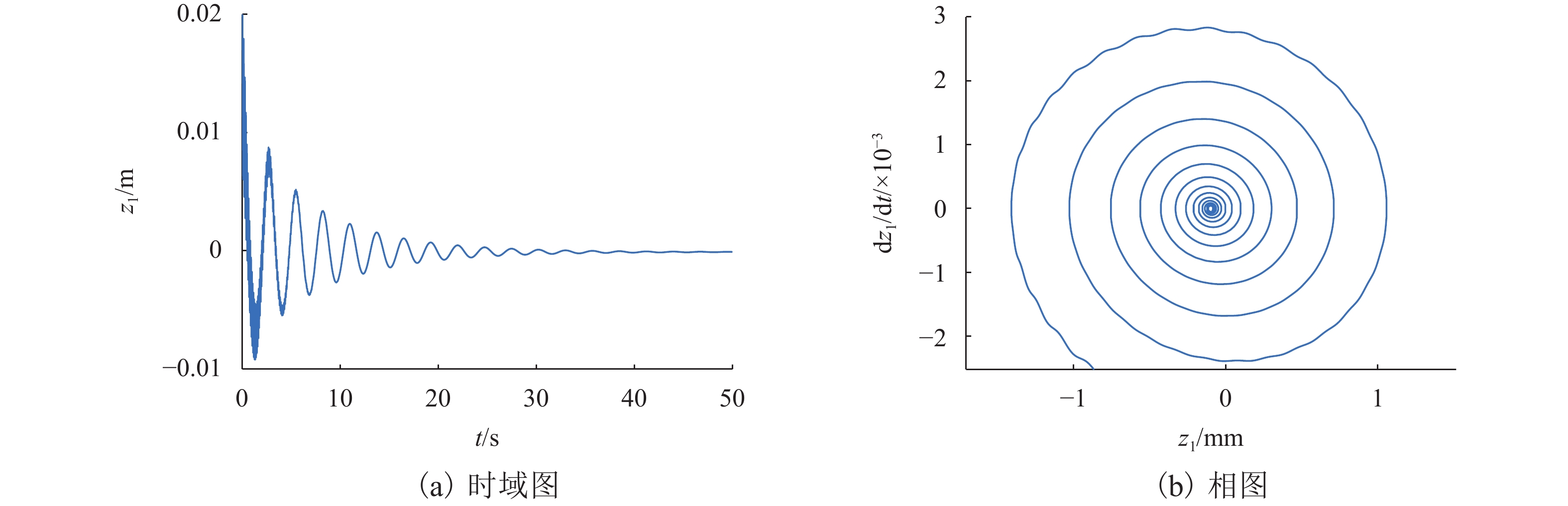
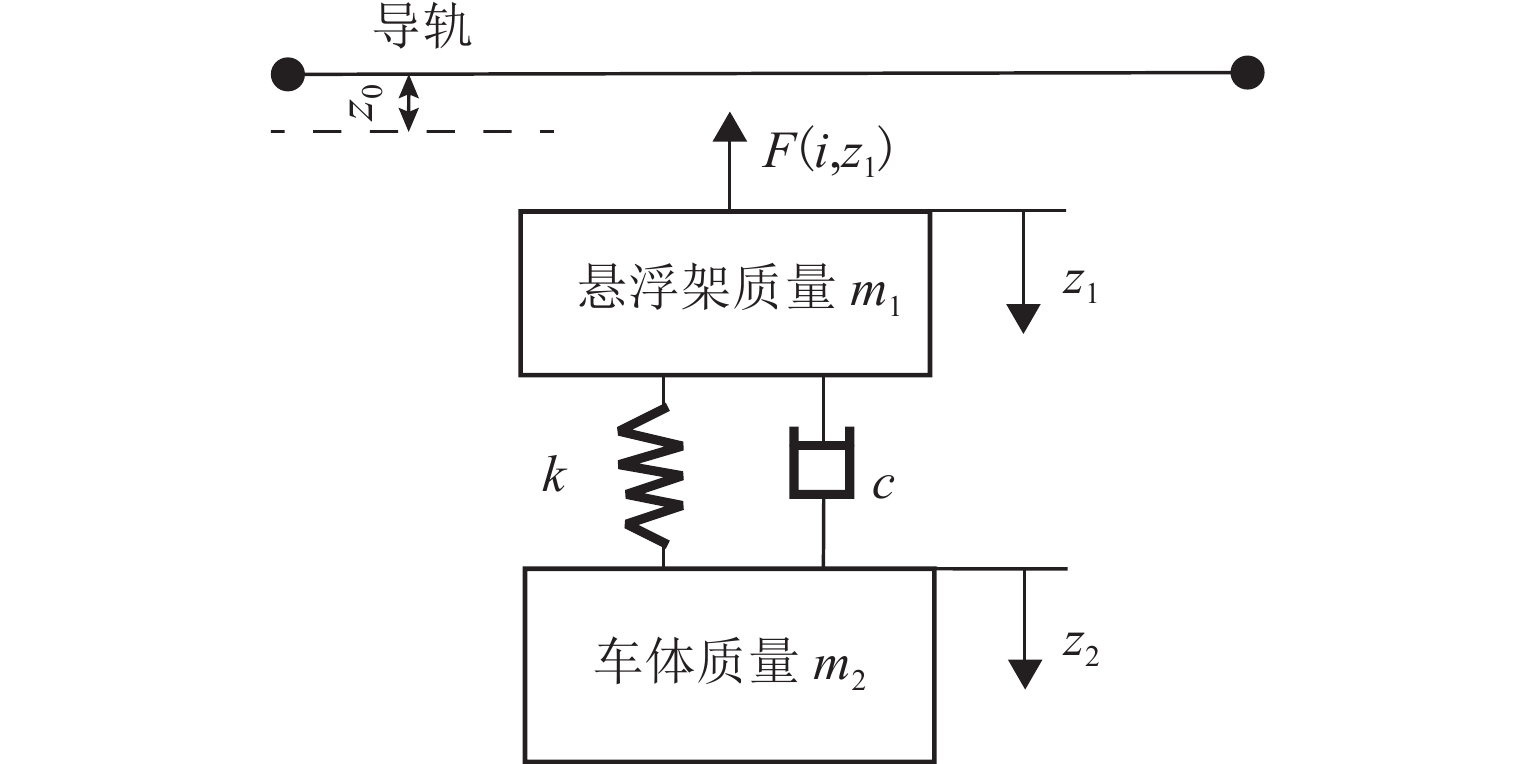
为研究控制器时滞对磁浮列车悬浮系统稳定性的影响，首先，以位移-速度为反馈控制参数，建立考虑控制器时滞的二自由度磁浮列车悬浮系统模型；其次，通过Routh-Hurwitz稳定性判据得到无时滞系统的稳定性区域，同时，依据特征根穿越虚轴边界条件，获得系统发生Hopf分岔的控制器时滞临界值；最后，分析反馈控制参数及系统参数与控制器时滞临界值的关系. 研究结果表明：当系统参数一定时，控制器时滞临界值随位移控制增益的增大而减小，随速度控制增益的增大先增大后减小；当反馈控制参数一定时，控制器时滞临界值随二系刚度的增大而减小，随二系阻尼的增大而增大；当系统时滞以10−6数量级在时滞临界值附近渐渐增大时，系统会从稳定—周期运动—不稳定逐渐变化，期间发生超临界Hopf分岔.
Abstract:In order to study the influence of controller time lag on the stability of the levitation system of the magnetic levitation train, firstly, the two-degree-of-freedom magnetic levitation train levitation system model is established by taking displacement-velocity as the feedback control parameter, and the controller time lag is taken into account; secondly, the stability region of the time lag-free system is obtained by the stability criterion of Routh-Hurwitz, meanwhile, based on the characteristic root crossing the imaginary axis boundary condition, we obtain the critical value of the time lag of the controller when the system undergoes Hopf bifurcation; finally, we analyze the relationship between the feedback control parameters and the system parameters and the critical value of the controller time lag. The results show that: when the system parameters are certain, the critical value of the controller time lag decreases with the increase of the displacement control gain, and increases and then decreases with the increase of the velocity control gain; when the feedback control parameters are certain, the critical value of the controller time lag decreases with the increase of the secondary suspension stiffness, and increases with the increase of the secondary suspension damping; as the time lag of the system increases asymptotically by an order of magnitude 10−6 around the critical value of time lag, the system will gradually change from stable-periodic motion-unstable, during which the supercritical Hopf bifurcation occurs.
-
Key words:
- magnetic levitation system /
- stability /
- time lag /
- feedback control /
- Hopf bifurcation
-
图 4 控制器时滞临界值与反馈控制参数的关系
Figure 4. Relationships between critical value of controller time lag and feedback control parameters
图 5 固有频率与反馈控制参数的关系
Figure 5. Relationships between natural frequency and feedback control parameters
图 6 控制器时滞临界值与系统参数的关系
Figure 6. Relationships between critical value of controller time lag and system parameters
图 7 固有频率与系统控制参数的关系
Figure 7. Relationships between natural frequency and system parameters
图 8 τ=0.000 900 s，τ<τ0时的系统响应
Figure 8. System response with time lag τ=0.000 900 s, τ<τ0
图 9 τ=0.001 000 s，τ>τ0时的系统响应
Figure 9. System response with time lag τ=0.001 000 s, τ>τ0
图 10 τ=0.000 986 s，τ>τ0时的系统响应
Figure 10. System response with time lag τ=0.000 986 s, τ>τ0
图 13 τ=0.000 900 s，τ <τ0时的系统响应
Figure 13. System response with time lag τ=0.000 900 s, τ<τ0
-
[1] 张舒,徐鉴. 时滞耦合系统非线性动力学的研究进展[J]. 力学学报,2017,49(3): 565-587.ZHANG Shu, XU Jian. Review on nonlinear dynamics in systems with coulpling delays[J]. Chinese Journal of Theoretical and Applied Mechanics, 2017, 49(3): 565-587. [2] 李帅,周继磊,任传波,等. 时变参数时滞减振控制研究[J]. 力学学报,2018,50(1): 99-108.LI Shuai, ZHOU Jilei, REN Chuanbo, et al. The research of time delay vibration control with time-varying parameters[J]. Chinese Journal of Theoretical and Applied Mechanics, 2018, 50(1): 99-108. [3] 王在华,胡海岩. 时滞动力系统的稳定性与分岔:从理论走向应用[J]. 力学进展,2013,43(1): 3-20.WANG Zaihua, HU Haiyan. Stability and bifurcation of delayed dynamic systems: from theory to application[J]. Advances in Mechanics, 2013, 43(1): 3-20. [4] 公徐路,许鹏飞. 含时滞反馈与涨落质量的记忆阻尼系统的随机共振[J]. 力学学报,2018,50(4): 880-889.GONG Xulu, XU Pengfei. Stochastic resonance of a memorial-damped system with time delay feedback and fluctuating mass[J]. Chinese Journal of Theoretical and Applied Mechanics, 2018, 50(4): 880-889. [5] TAFFO G I K, SIEWE M S, TCHAWOUA C. Stability switches and bifurcation in a two-degrees-of-freedom nonlinear quarter-car with small time-delayed feedback control[J]. Chaos, Solitons & Fractals, 2016, 87(1): 226-239. [6] 马卫华,胡俊雄,李铁,等. EMS型中低速磁浮列车悬浮架技术研究综述[J]. 西南交通大学学报,2023,58(4): 20-733.MA Weihua, HU Junxiong, LI Tie, et al. Technologies research review of electro-magnetic suspension medium-low-speed maglev train levitation frame[J]. Journal of Southwest Jiaotong University, 2023, 58(4): 20-733. [7] LEE H W, KIM K C, LEE J. Review of maglev train technologies[J]. IEEE Transactions on Magnetics, 2006, 42(7): 1917-1925. doi: 10.1109/TMAG.2006.875842 [8] 刘士苋,王磊,王路忠,等. 电动悬浮列车及车载超导磁体研究综述[J]. 西南交通大学学报,2023,58(4): 734-753.LIU Shixian, WANG Lei, WANG Luzhong, et al. Review on electrodynamic suspension trains and on-board superconducting magnets[J]. Journal of Southwest Jiaotong University, 2023, 58(4): 734-753. [9] ZHANG L L, HUANG L H, ZHANG Z Z. Stability and Hopf bifurcation of the maglev system with delayed position and speed feedback control[J]. Nonlinear Dynamics, 2009, 57(1): 197-207. [10] YAU J D. Response of a maglev vehicle moving on a two-span flexible guideway[J]. Journal of Mechanics, 2010, 26(1): 95-103. doi: 10.1017/S1727719100003762 [11] ZHANG Z Z, ZHANG L L. Hopf bifurcation of time-delayed feedback control for maglev system with flexible guideway[J]. Applied Mathematics and Computation, 2013, 219(11): 6106-6112. doi: 10.1016/j.amc.2012.12.045 [12] 王洪坡. EMS型低速磁浮列车/轨道系统的动力相互作用问题研究[D]. 长沙:国防科学技术大学,2007. [13] 翟婉明,赵春发. 磁浮车辆/轨道系统动力学(Ⅰ)——磁/轨相互作用及稳定性[J]. 机械工程学报,2005,41(7): 1-10. doi: 10.3901/JME.2005.07.001ZHAI Wanming, ZHAO Chunfa. Dynamics of maglev vehicle/ guideway systems(I)—Magnet/rail interaction and system stability[J]. Journal of Mechanical Engineering, 2005, 41(7): 1-10. doi: 10.3901/JME.2005.07.001 [14] DONG H, ZENG J, XIE J H, et al. Bifurcation\instability forms of high speed railway vehicles[J]. Science China Technological Sciences, 2013, 56(7): 1685-1696. doi: 10.1007/s11431-013-5254-x [15] 苏红建. 基于车路耦合磁悬浮列车非线性振动控制研究[D]. 淄博:山东理工大学,2022. [16] XU J Q, CHEN C, GAO D G, et al. Nonlinear dynamic analysis on maglev train system with flexible guideway and double time-delay feedback control[J]. Journal of Vibroengineering, 2017, 19(8): 6346-6362. doi: 10.21595/jve.2017.18970 [17] 陈晓昊,马卫华. 控制器时滞对磁浮系统稳定性影响分析[J]. 机车电传动,2019(2): 139-143,147.CHEN Xiaohao, MA Weihua. Analysis on the effect of controller time delay on the stability of maglev system[J]. Electric Drive for Locomotives, 2019(2): 139-143,147. [18] LI J H, LI J, ZHOU D F, et al. Self-excited vibration problems of maglev vehicle-bridge interaction system[J]. Journal of Central South University, 2014, 21(11): 4184-4192. doi: 10.1007/s11771-014-2414-5 [19] 王洪坡,李杰. 一类非自治位置时滞反馈控制系统的亚谐共振响应[J]. 物理学报,2007,56(5): 2504-2516. doi: 10.7498/aps.56.2504WANG Hongpo, LI Jie. Sub-harmonic resonances of the non-autonomous system with delayed position feedback control[J]. Acta Physica Sinica, 2007, 56(5): 2504-2516. doi: 10.7498/aps.56.2504 [20] WANG H P, LI J, ZHANG K. Stability and Hopf bifurcation of the maglev system with delayed speed feedback control[J]. Acta Automatica Sinica, 2007, 33(8): 829-834. doi: 10.1360/aas-007-0829 [21] WANG H P, LI J, ZHANG K. Non-resonant response, bifurcation and oscillation suppression of a non-autonomous system with delayed position feedback control[J]. Nonlinear Dynamics, 2008, 51(3): 447-464. [22] WANG H P, LI J, ZHANG K. Sup-resonant response of a nonautonomous maglev system with delayed acceleration feedback control[J]. IEEE Transactions on Magnetics, 2008, 44(10): 2338-2350. [23] 吴晗,曾晓辉,史禾慕. 考虑间隙反馈控制时滞的磁浮车辆稳定性研究[J]. 力学学报,2019,51(2): 550-557.WU Han, ZENG Xiaohui, SHI Hemu. Stability analysis of maglev vehicle with delayed position feedback control[J]. Chinese Journal of Theoretical and Applied Mechanics, 2019, 51(2): 550-557. [24] 沈飞,武建军. 时滞反馈磁浮控制系统的周期运动稳定性分析[J]. 兰州大学学报(自然科学版),2008,44(5): 131-136.SHEN Fei, WU Jianjun. Stability analysis of periodic motion of the maglev system with delayed velocity feedback control[J]. Journal of Lanzhou University (Natural Sciences), 2008, 44(5): 131-136. [25] SUN Y G, XU J Q, CHEN C, et al. Reinforcement learning-based optimal tracking control for levitation system of maglev vehicle with input time delay[J]. IEEE Transactions on Instrumentation Measurement, 2022, 71(8): 3142059.1-3142059.13. [26] ZHANG L L, HUANG L H, ZHANG Z Z. Hopf bifurcation of the maglev time-delay feedback system via pseudo-oscillator analysis[J]. Mathematical and Computer Modelling, 2010, 52(5/6): 667-673. [27] CUI X K, LI H L, ZHANG L, et al. Complete synchronization for discrete-time fractional-order coupled neural networks with time delays[J]. Chaos, Solitons & Fractals, 2023, 174:113772.1-113772.8. [28] FENG Y H, HU L J. On the quasi-controllability of continuous-time dynamic fuzzy control systems[J]. Chaos, Solitons & Fractals, 2006, 30(1): 177-188. [29] DE OLIVEIRA EVALD P J D, HOLLWEG G V, TAMBARA R V, et al. Lyapunov stability analysis of a robust model reference adaptive PI controller for systems with matched and unmatched dynamics[J]. Journal of the Franklin Institute, 2022, 359(13): 6659-6689. doi: 10.1016/j.jfranklin.2022.07.014 [30] SOLGI Y, FATEHI A, NIKOOFARD A, et al. Design of optimal PID controller for multivariable time-varying delay discrete-time systems using non-monotonic Lyapunov-Krasovskii approach[J]. Journal of the Franklin Institute, 2021, 358(13): 6634-6665. doi: 10.1016/j.jfranklin.2021.06.026 [31] BORASE R P, MAGHADE D K, SONDKAR S Y, et al. A review of PID control, tuning methods and applications[J]. International Journal of Dynamics and Control, 2021, 9(2): 818-827. doi: 10.1007/s40435-020-00665-4 [32] 陈志贤,李忠继,杨吉忠,等. 常导高速电磁悬浮车辆二系悬挂结构对比优化[J]. 机械工程学报,2022,58(10): 160-168,179. doi: 10.3901/JME.2022.10.160CHEN Zhixian, LI Zhongji, YANG Jizhong, et al. Comparison and optimization of secondary suspension structure of high speed EMS vehicle[J]. Journal of Mechanical Engineering, 2022, 58(10): 160-168,179. doi: 10.3901/JME.2022.10.160 [33] WU H, ZENG X H, GAO D G, et al. Dynamic stability of an electromagnetic suspension maglev vehicle under steady aerodynamic load[J]. Applied Mathematical Modelling, 2021, 97: 483-500. doi: 10.1016/j.apm.2021.04.008 [34] 黎松奇,张昆仑,陈殷,等. 弹性轨道上磁浮车辆动力稳定性判断方法[J]. 交通运输工程学报,2015,15(1): 43-49.LI Songqi, ZHANG Kunlun, CHEN Yin, et al. Judgment method of maglev vehicle dynamic stability on flexible track[J]. Journal of Traffic and Transportation Engineering, 2015, 15(1): 43-49. [35] 吴晗,曾晓辉. 气动升力下磁浮车辆非线性响应研究[J]. 机械工程学报,2021,57(14): 223-231. doi: 10.3901/JME.2021.14.223WU Han, ZENG Xiaohui. Nonlinear dynamics of maglev vehicle under aerodynamic lift[J]. Journal of Mechanical Engineering, 2021, 57(14): 223-231. doi: 10.3901/JME.2021.14.223 [36] 张继业,杨翊仁,曾京. Hopf分岔的代数判据及其在车辆动力学中的应用[J]. 力学学报,2000,32(5): 596-605.ZHANG Jiye, YANG Yiren, ZENG Jing. An algorithm crterion for Hopf bifurcation and its applications in vehicle dynamics[J]. Acta Mechanica Sinica, 2000, 32(5): 596-605. [37] CHEN X H, MA W H, LUO S H. Study on stability and bifurcation of electromagnet-track beam coupling system for EMS maglev vehicle[J]. Nonlinear Dynamics, 2020, 101(4): 2181-2193. doi: 10.1007/s11071-020-05917-8 [38] 张玲玲. 磁浮列车悬浮系统的Hopf分岔及滑模控制研究[D]. 长沙:湖南大学,2010. [39] 梁鑫. 磁浮列车车轨耦合振动分析及试验研究[D]. 成都:西南交通大学,2015. -
下载:
点击查看大图
计量
- 文章访问数: 344
- HTML全文浏览量: 99
- PDF下载量: 66
- 被引次数: 0