Nonlinear Buckling Analysis of Suspended Domes Considering Initial Curvature of Members
-
摘要:
为揭示杆件初弯曲对弦支穹顶结构稳定承载力的影响规律,以多段直梁法模拟杆件初弯曲,采用随机缺陷模态法引入不同形状及幅值的杆件初弯曲,对弦支穹顶结构进行非线性屈曲分析;引入整体缺陷与杆件初弯曲,考察2种缺陷的共同施加对结构稳定性能的影响. 结果表明:仅考虑杆件初弯曲时,弦支穹顶结构的稳定承载力系数平均值显著降低(最大降幅为33.84%),该结构对杆件初弯曲较为敏感;相比于正弦全波,以正弦半波为初弯曲形状来引入杆件初弯曲,对结构的稳定性更为不利;相比于理想结构,同时考虑整体缺陷与杆件初弯曲时,结构的稳定承载力系数进一步降低(最大降幅为44.80%),但其降幅小于两者分别引入的降幅之和,2种缺陷的同时施加,对结构的稳定承载力存在耦合影响,一定程度上削弱了两者单独引入时的不利影响.
Abstract:In order to reveal the influence laws of the initial curvature of members on the stability bearing capacity of suspended domes, a nonlinear buckling analysis of suspended domes was carried out by applying the multi-beam method to simulate the initial curvature of members and the random imperfection mode method to introduce the initial curvature of members with different shapes and amplitudes. The overall imperfection and the initial curvature of members were introduced to investigate the effect of the two kinds of imperfections imposed jointly on the structural stability behaviors. The results show that the mean coefficients of stability bearing capacity of suspended domes are significantly reduced when only the initial curvature of members is considered, and the maximum reduction is 33.84%, which indicates that the structure is sensitive to the initial curvature of members. Compared with the sinusoidal full-wave, the sinusoidal half-wave as the shape of initial curvature is more unfavorable to the structural stability. When the overall imperfection and the initial curvature of members are both considered, the coefficients of stability bearing capacity are further reduced for the suspended domes compared with the perfect structure, with the maximum reduction being 44.80%, but the reductions are smaller than the sums of reductions when the two kinds of imperfections are introduced separately. The joint action of the two kinds of imperfections has coupling effects on the structural stability bearing capacity, which weakens the adverse effects when the two kinds of imperfections are introduced separately to some extent.
-
弦支穹顶[1]是由上部单层网壳和下部索杆体系组合而成的新型杂交空间结构体系,刚柔相济,受力合理,近年来,在大跨度屋盖结构中得到广泛应用[2-3]. 但该结构的矢跨比较小,静力稳定性能是其设计选型的关键因素之一[4].
文献[5]以河北北方学院体育馆弦支穹顶为对象,考虑活载不利布置、初始几何缺陷分布模式和大小等因素,探讨其对结构稳定承载力的影响. 文献[6]采用逆推求解法,得到800 m凯威特巨型网格弦支穹顶的最不利屈曲模态及其阶数. 文献[7]考虑几何非线性、初始几何缺陷及活载的半跨布置,对厚街体育馆弦支穹顶与相应单层网壳的非线性屈曲性能进行了对比研究. 在此基础上,考察初始几何缺陷分布模式及材料弹塑性对弦支穹顶稳定承载力的影响,以此探讨一致缺陷模态法的适用性. 文献[8]引入节点安装偏差、杆件初偏心及索的预应力偏差,并考虑材料弹性模量的变化,提出适用于弦支穹顶稳定性分析的随机缺陷法. 文献[9]提出以N阶特征缺陷模态法作为弦支穹顶初始几何缺陷的施加方法,并给出弹性全过程分析的稳定安全系数取值. 文献[10]对跨度为108 m的弦支穹顶进行弹塑性屈曲分析,提出梁单元的划分方法,考察杆件初弯曲对结构稳定承载力的影响,结果表明,该法可较精确地模拟杆件初弯曲,且计算量增加不大. 文献[11]探讨了杆件初弯曲的分布模式及幅值对单层球壳稳定性的影响,并给出杆件初弯曲幅值的建议取值. 文献[12]对半刚性连接单层网壳进行稳定性分析,考察杆件初弯曲、节点安装偏差及节点刚度对结构稳定性能的影响. 文献[13]指出,H型钢杆件沿弱轴方向的初弯曲对H型钢弦支穹顶的稳定承载力影响较大,因此,该类杆件加工时,应尽量避免沿弱轴方向的初弯曲.
目前,对弦支穹顶结构的缺陷稳定性研究主要集中于整体缺陷(节点安装偏差),而对杆件缺陷(重点针对初弯曲)的研究相对较少,且相关工作尚未涉及这2种缺陷的同时施加对该结构稳定承载力的耦合影响分析. 因此,对考虑杆件初弯曲的弦支穹顶结构开展静力稳定性研究具有重要的科学意义. 为此,本文以多段直梁法模拟杆件初弯曲,对理想结构引入杆件初弯曲,并进行弹塑性全过程分析,探讨不同形状及幅值的杆件初弯曲对弦支穹顶结构非线性屈曲性能的影响程度. 在此基础上,对整体缺陷结构引入杆件初弯曲,考察2种缺陷的共同施加对该结构稳定性能的耦合影响,所得结论可为类似结构的分析与设计提供理论依据.
1. 杆件初弯曲模拟方法
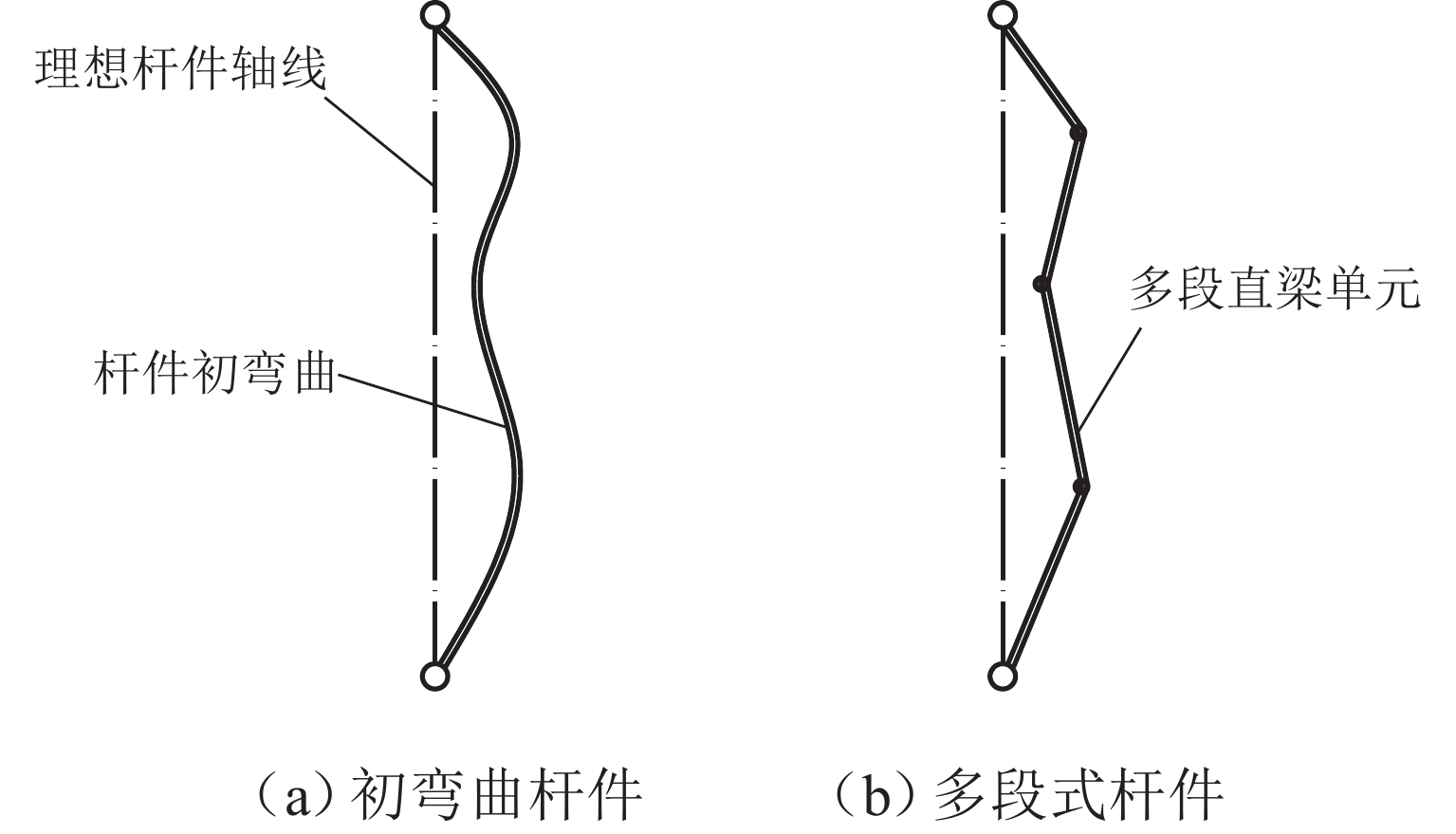
杆件初弯曲是工程实践中难以避免的初始缺陷之一,在轴心压力的作用下,可产生对杆件不利的附加弯矩,进而影响结构的稳定性能. 杆件的初弯曲特征包括初弯曲形状、初弯曲幅值及初弯曲方向角,其空间弯曲示意如图1所示. 图中:i、j均为杆端节点编号,δ为初弯曲幅值,β为初弯曲方向角. 以端节点i为原点O,杆件ij的理想轴线为X轴,设定杆件的局部坐标系为O-XYZ. 在三维空间中定义一个参考点A,以Aij为参考面,其与杆件初弯曲平面的夹角定义为β,逆时针为正.
当理想直杆发生初弯曲后,曲杆上某一点偏离X轴的距离为
d=δf(x), (1) 式中:f(x)为初弯曲形状,x为理想直杆上某一点到原点O的距离.
依据初弯曲形状f(x)、幅值δ及方向角β可定量表征杆件的初弯曲. 假定(x0,y0,z0)、(x1,y1,z1)分别为理想直杆中的某一点及其发生初弯曲后的坐标,两者满足
{x1=x0,y1=y0+δf(x0)sinβ,z1=z0+δf(x0)cosβ. (2) 本文采用文献[14]提出的多段直梁法来模拟杆件的初弯曲,如图2所示. 多段直梁法即用多段直梁单元形成多段式折线,以此模拟杆件的初弯曲形状. 先将理想直杆打断为k段,再由式(2)计算其发生初弯曲后内部k−1个节点的坐标,并重新依次连接各节点,即可模拟杆件的初弯曲.
杆件初弯曲形状f(x)一般可依据理想直杆的屈曲模态选取,而β及δ往往无法预测,可在特定区间以随机采样的方法确定. 其中,β可假定在[0∘, 360∘)区间服从均匀分布,即β ~U[0∘, 360∘);对δ,考虑到相关规范对杆件平直度的要求,可假定其满足极值Ⅰ型分布[15],相应的分布函数为
F(δ)=1−e−ev(δ−q), (3) 式中:F(δ)为初弯曲幅值δ的分布函数,q为分布的众值,v为偏度的量测.
若假定杆件初弯曲的最大幅值为u,出现大于此值的概率为2.5%,且δ为0的概率为1.0%,则有
P(δ>u)=1−F(u)=2.5%, (4) F(0)=1.0%. (5) 联立式(4)、(5),可得q=0.78u,v=5.9/u.
以上述概率分布随机生成一系列初弯曲幅值δ时,有极小的概率生成小于0的数. 在此设定,当随机生成的数小于0时,令该数为0.
2. 分析模型
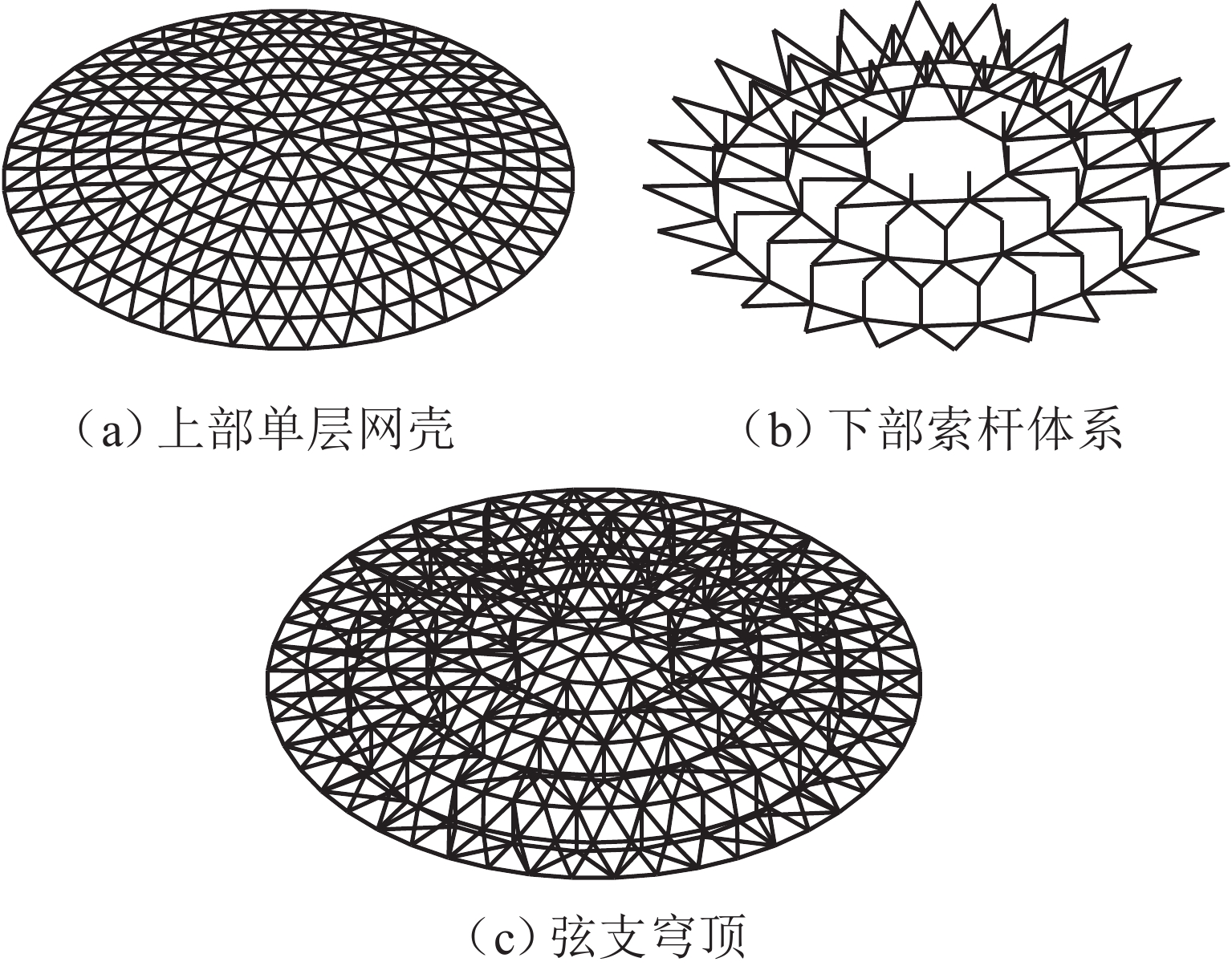
基于某实际工程,建立跨度为80 m、矢跨比为1/10的凯威特-联方型弦支穹顶分析模型(图3). 结构的频数为8,其中,凯威特部分为6圈,联方部分为2圈,且下部设置3圈索杆体系. 环向索的初始预应力由内至外分别为102.828、529.749、
1607.767 kN,其确定方法详见文献[16],限于篇幅,在此不再赘述. 构件和材料规格如表1所示.表 1 构件和材料规格Table 1. Specifications of members and materials结构部位 构件 材质 规格 上部单层
网壳凯威特
部分径向杆 Q355B ϕ219 × 12 环向杆 Q355B ϕ219 × 12 斜杆 Q355B ϕ203 × 10 联方
部分环向杆 Q355B ϕ203 × 10 斜杆 Q355B ϕ194 × 10 撑杆 Q355B ϕ168 × 8 下部索杆
体系环向索 内圈 平行钢丝
束,1670 级ϕ5 × 61 中圈 平行钢丝
束,1670 级ϕ5 × 91 外圈 平行钢丝
束,1670 级ϕ5 × 139 径向索 平行钢丝
束,1670 级ϕ5 × 55 选用稀索体系弦支穹顶,各圈撑杆由内至外的高度分别为4.9、5.7、6.6 m. 上部单层网壳杆件及撑杆的弹性模量均为206 × 103 N/mm2,拉索的弹性模量为195 × 103 N/mm2,屋面附加恒载取0.5 kN/m2,不上人屋面活载取0.5 kN/m2(全跨布置),以等效节点荷载的形式作用于上部单层网壳,荷载组合为1.0恒载 + 1.0活载的标准组合. 采用通用有限元软件ANSYS进行分析,上部单层网壳节点刚接,其杆件、撑杆、拉索分别采用Beam188单元、Link8单元、Link10单元模拟,与外圈径向索相连的周边节点的边界条件为固定铰支座.
3. 理想结构引入杆件初弯曲
文献[17]研究表明,采用多段直梁法模拟杆件初弯曲时,将杆件划分为4段等长直线梁单元,即可满足计算精度要求. 鉴于弦支穹顶结构的缺陷敏感区域主要位于上部单层网壳[18],在此,本文将该区域的杆件沿杆长均匀划分为4段直线梁单元,以此对弦支穹顶结构进行仅考虑杆件初弯曲的非线性屈曲分析.
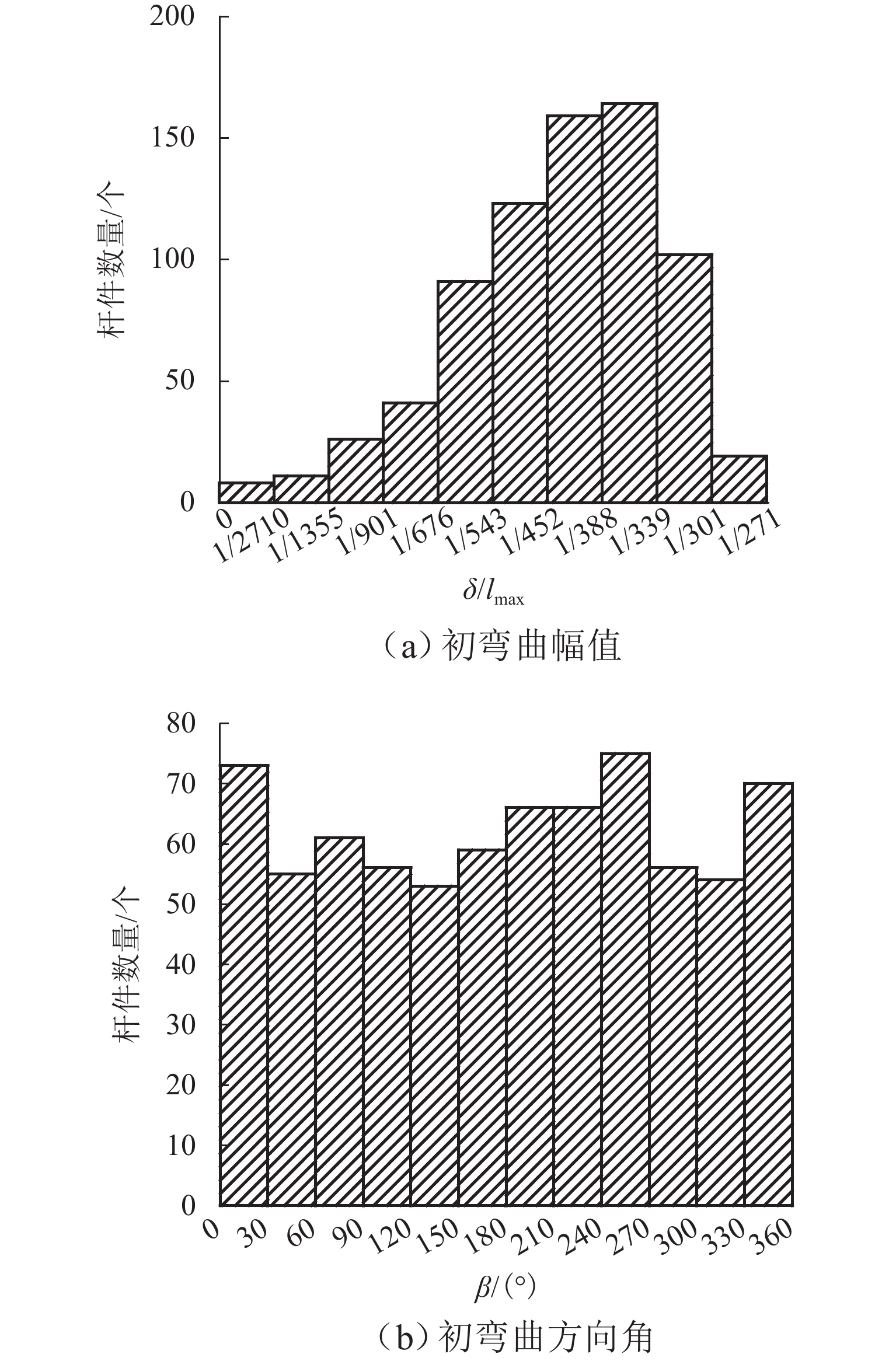
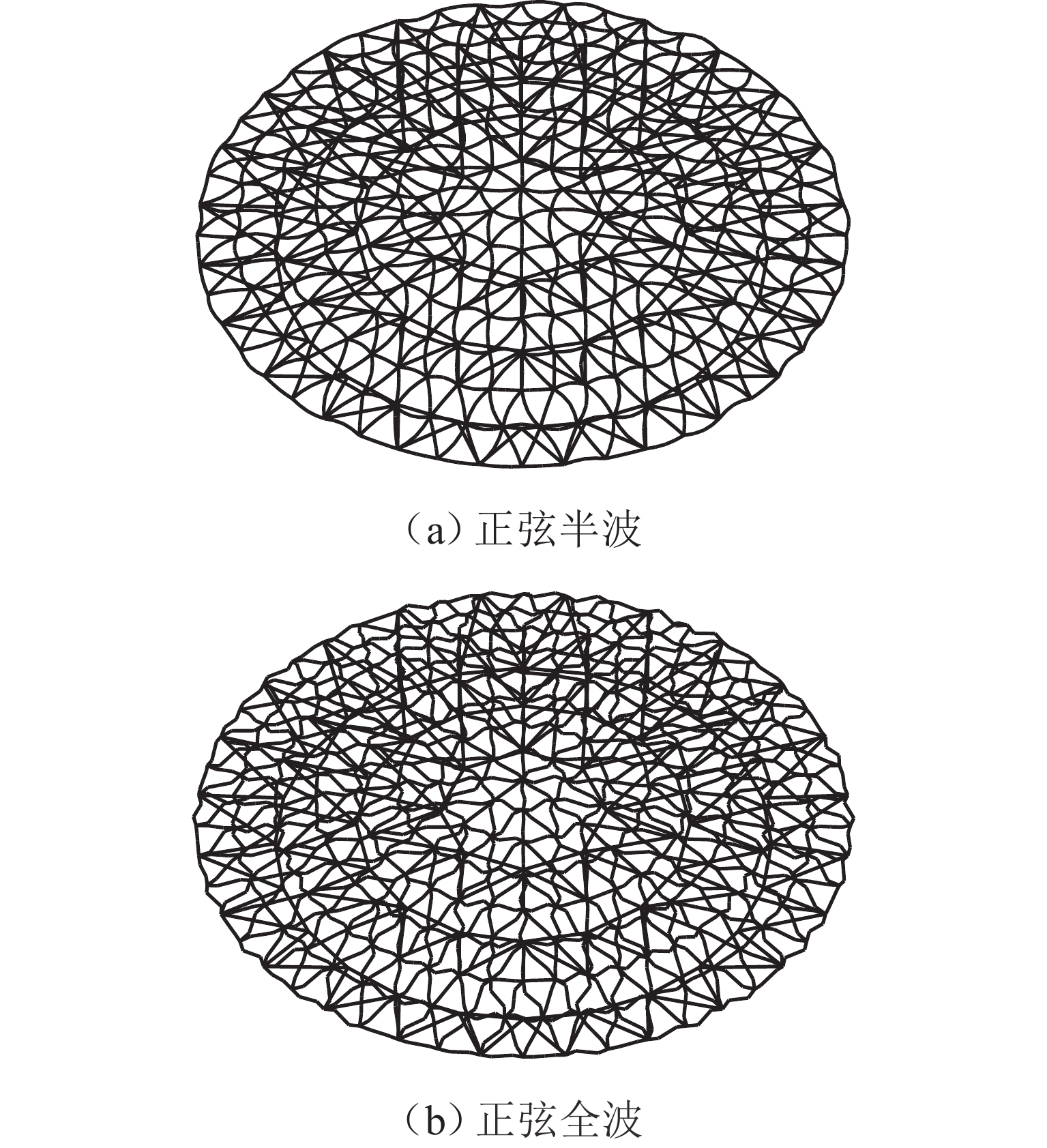
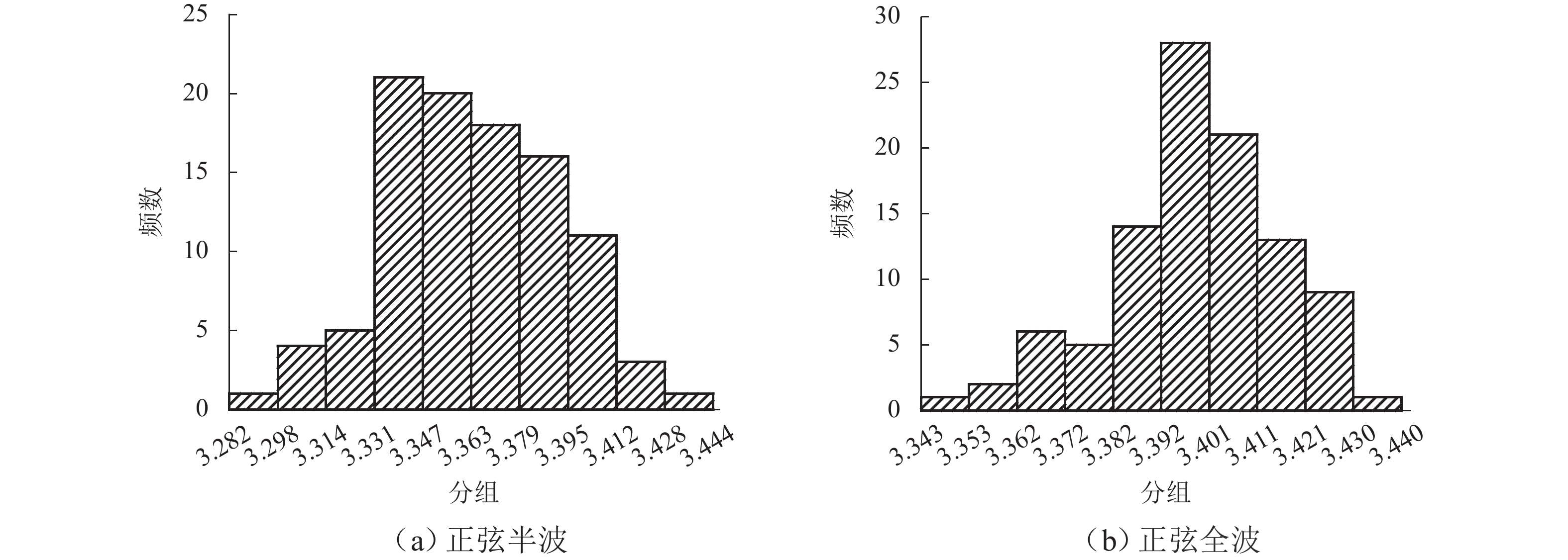
采用随机缺陷模态法,对上述模型引入杆件初弯曲,样本容量取100. 其中,杆件初弯曲形状分别取正弦半波f(x)=sin(πx/l)及正弦全波f(x)=sin(2πx/l). 初弯曲幅值最大值分别取lmax/300、lmax/400、lmax/500、lmax/600及lmax/700. 图4为一次模拟所得的初弯曲幅值(以最大值取lmax/300为例)及方向角分布直方图. 其中,lmax为单一模型上部单层网壳中所有杆件长度的最大值. 图5为理想结构引入杆件初弯曲后(初弯曲幅值最大值均为lmax/300)的模型图.
限于篇幅,在此仅列出以lmax/300为初弯曲幅值最大值,且分别以正弦半波、正弦全波为初弯曲形状,对理想结构引入杆件初弯曲并进行双重非线性分析计算(考虑材料非线性时,采用两段式理想弹塑性本构关系模型),结果如表2所示,其对应的分布直方图如图6所示. 表中:Kmax、Kmin、μ、σ分别为稳定承载力系数的最大值、最小值、平均值和标准差.
表 2 仅考虑杆件初弯曲的稳定承载力系数Table 2. Coefficients of stability bearing capacity considering initial curvature of members only初弯曲形状 稳定承载力系数 正弦半波 3.361,3.328,3.382,3.390,3.391,3.398,
3.377,3.345,3.338,3.355,3.343,3.367,
3.324,3.401,3.344,3.368,3.371,3.353,
3.375,3.350,3.387,3.379,3.340,3.389,
3.389,3.340,3.320,3.313,3.378,3.401,
3.282,3.378,3.404,3.369,3.370,3.331,
3.444,3.371,3.333,3.342,3.310,3.361,
3.394,3.402,3.338,3.314,3.377,3.348,
3.337,3.359,3.383,3.405,3.358,3.351,
3.360,3.383,3.391,3.329,3.349,3.421,
3.365,3.414,3.346,3.386,3.354,3.339,
3.357,3.393,3.418,3.364,3.339,3.361,
3.364,3.339,3.362,3.345,3.384,3.348,
3.346,3.336,3.356,3.403,3.346,3.350,
3.409,3.375,3.379,3.393,3.377,3.326,
3.355,3.312,3.336,3.397,3.382,3.351,
3.332,3.404,3.397,3.385Kmin, {K_{\max }} = 3.444 , \mu = 3.3642 , {\sigma } = 0.028\;8 正弦全波 3.424,3.416,3.406,3.416,3.395,3.387,
3.409,3.357,3.400,3.415,3.410,3.392,
3.406,3.395,3.370,3.369,3.390,3.409,
3.380,3.399,3.370,3.400,3.413,3.422,
3.398,3.385,3.405,3.422,3.424,3.423,
3.377,3.401,3.392,3.403,3.421,3.360,
3.408,3.393,3.407,3.411,3.397,3.440,
3.406,3.390,3.392,3.410,3.381,3.391,
3.411,3.397,3.388,3.397,3.409,3.419,
3.388,3.409,3.403,3.366,3.372,3.388,
3.410,3.399,3.367,3.386,3.427,3.412,
3.394,3.401,3.411,3.402,3.383,3.393,
3.400,3.415,3.392,3.427,3.398,3.421,
3.393,3.373,3.387,3.390,3.409,3.395,
3.384,3.395,3.406,3.414,3.401,3.416,
3.343,3.413,3.376,3.406,3.395,3.394,
3.400,3.410,3.387,3.410{K_{\min }} = 3.343 , {K_{\max }} = 3.440 , \mu = 3.3987 , {\sigma } = 0.017\;0 由表2和图6可见:理想结构引入杆件初弯曲后,由随机缺陷模态法分析所得的稳定承载力系数分布直方图呈现中间高、两边低的特征;当初弯曲形状分别为正弦半波、正弦全波时,其对应的稳定承载力系数最小值与最大值之差均小于5%. 由此表明,当初弯曲幅值最大值确定时,理想结构引入不同初弯曲分布模式后,稳定承载力系数变化幅度不明显. 对表2中的两组数据分别进行χ2拟合优度检验,具体计算过程详见文献[16]. 结果表明,两组数据均服从正态分布,其对应保证率为99.87% ( 3\sigma 原则)的稳定承载力系数分别为
3.2778 、3.3477. 此外,查询计算结果,未引入杆件初弯曲时,理想结构相应的稳定承载力系数为5.0850 . 与其相比,引入杆件初弯曲后,结构的稳定承载力系数分别降低35.54%及34.17%,降幅显著,表明该类弦支穹顶结构对杆件初弯曲较为敏感.结构在不同初弯曲形状、初弯曲幅值最大值下的稳定承载力系数对比如表3所示. 鉴于部分计算结果不满足χ2检验,因此,下文以平均值进行对比,不同参数下稳定承载力系数平均值对比如图7所示.
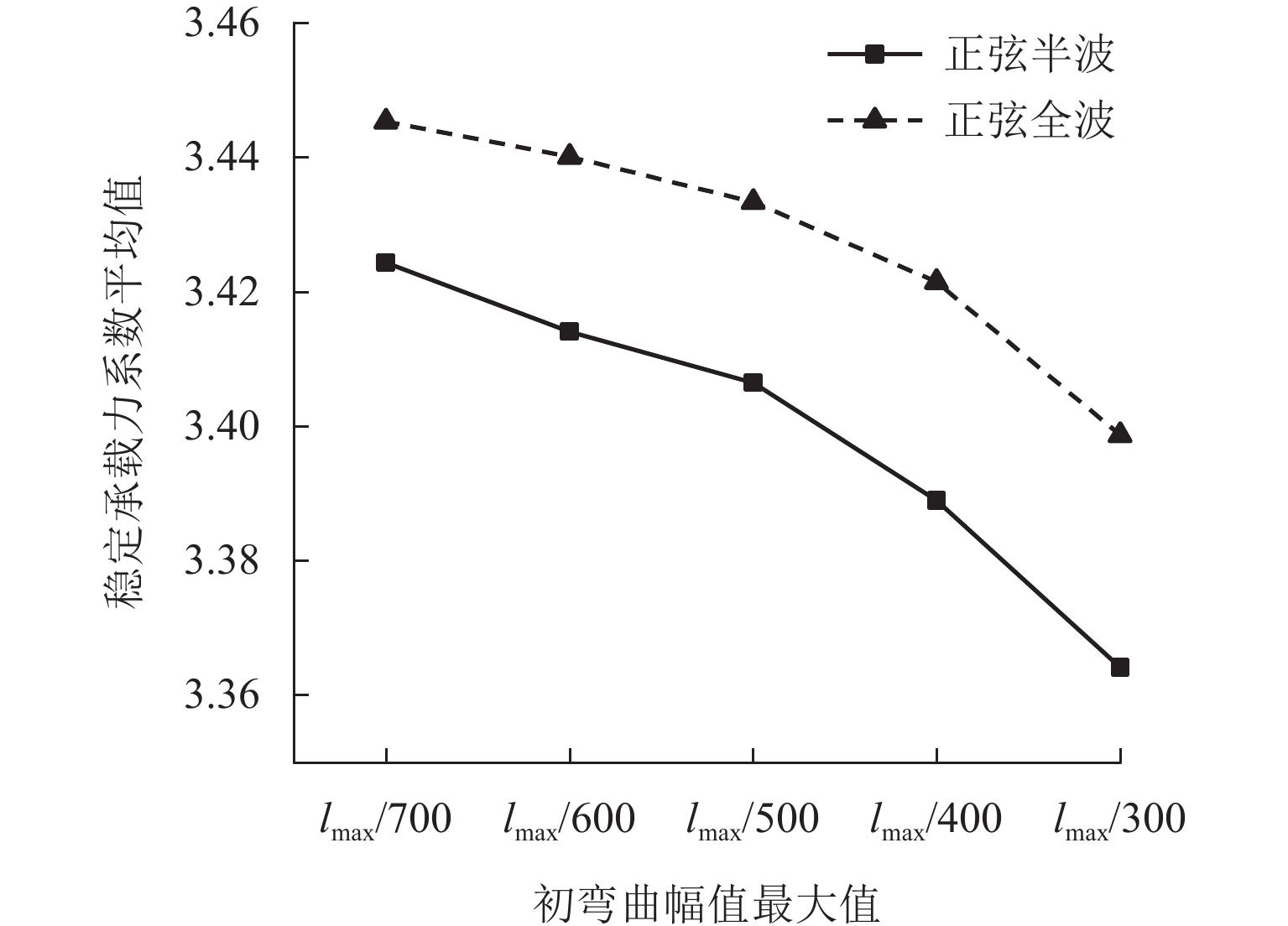
表 3 仅考虑杆件初弯曲的稳定承载力系数对比Table 3. Comparison of coefficients of stability bearing capacity considering initial curvature of members only初弯曲幅值最大值 初弯曲形状 Kmin Kmax \mu \sigma 是否满足 χ2 检验 \mu - 3\sigma lmax/700 正弦半波 3.365 3.482 3.4243 0.0178 否 正弦全波 3.424 3.473 3.4453 0.0092 是 3.4177 lmax/600 正弦半波 3.344 3.469 3.4141 0.0216 是 3.3493 正弦全波 3.400 3.465 3.4401 0.0102 是 3.4095 lmax/500 正弦半波 3.356 3.461 3.4065 0.0192 是 3.3489 正弦全波 3.401 3.459 3.4334 0.0109 是 3.4007 lmax/400 正弦半波 3.305 3.476 3.3890 0.0305 是 3.2975 正弦全波 3.369 3.443 3.4215 0.0120 是 3.3855 lmax/300 正弦半波 3.282 3.444 3.3642 0.0288 是 3.2778 正弦全波 3.343 3.440 3.3987 0.0170 是 3.3477 由表3及图7可见,当初弯曲幅值最大值相同且以正弦半波为初弯曲形状时,所得的稳定承载力系数平均值均略低于正弦全波. 究其原因,主要是由于杆件以正弦半波为初弯曲形状时,其引起的附加弯矩使杆件产生同向曲率,而以正弦全波为初弯曲形状时,其引起的附加弯矩使杆件产生异向曲率,且前者所引起的杆件弯曲应力更大,因而对结构的稳定性更为不利. 基于此,下文分析中,杆件初弯曲形状均取正弦半波. 此外,不同初弯曲形状下,随着初弯曲幅值最大值的增大,结构的稳定承载力系数平均值均依次减小,且相比于理想结构,其降幅均超过30.00%,最大为33.84%,再次表明该类弦支穹顶结构对杆件初弯曲较为敏感. 工程实践中,对类似结构的稳定性分析建议考虑杆件初弯曲的影响.
4. 整体缺陷结构引入杆件初弯曲
鉴于实际工程中整体缺陷(节点安装偏差)与杆件初弯曲往往同时存在,因此,有必要考察两者的共同施加对弦支穹顶结构非线性屈曲性能的影响. 仍以图3所示的分析模型为对象,同时考虑整体缺陷与杆件初弯曲,对其进行双重非线性分析. 其中,整体缺陷最大值取结构跨度的1/300,且分别以随机缺陷及特征缺陷为整体缺陷分布模式,其确定过程详见文献[16],对应的弹塑性稳定承载力系数分别为4.033、3.518,均满足相关规范[19]规定的不小于2.0的要求. 此外,与上文分析一致,仅对上部单层网壳区域引入整体缺陷和杆件初弯曲,初弯曲幅值最大值分别取lmax/300、lmax/400、lmax/500、lmax/600及lmax/700,样本容量同样均取100.
分别对上述不同缺陷分布模式(随机缺陷、特征缺陷)的整体缺陷结构引入杆件初弯曲,限于篇幅,图8仅列出了杆件初弯曲形状均取正弦半波、初弯曲幅值最大值均取lmax/300的模型图.
采用随机缺陷模态法,对同时考虑整体缺陷与杆件初弯曲的各模型进行双重非线性分析. 不同参数下的稳定承载力系数对比如表4所示,其平均值对比如图9所示.
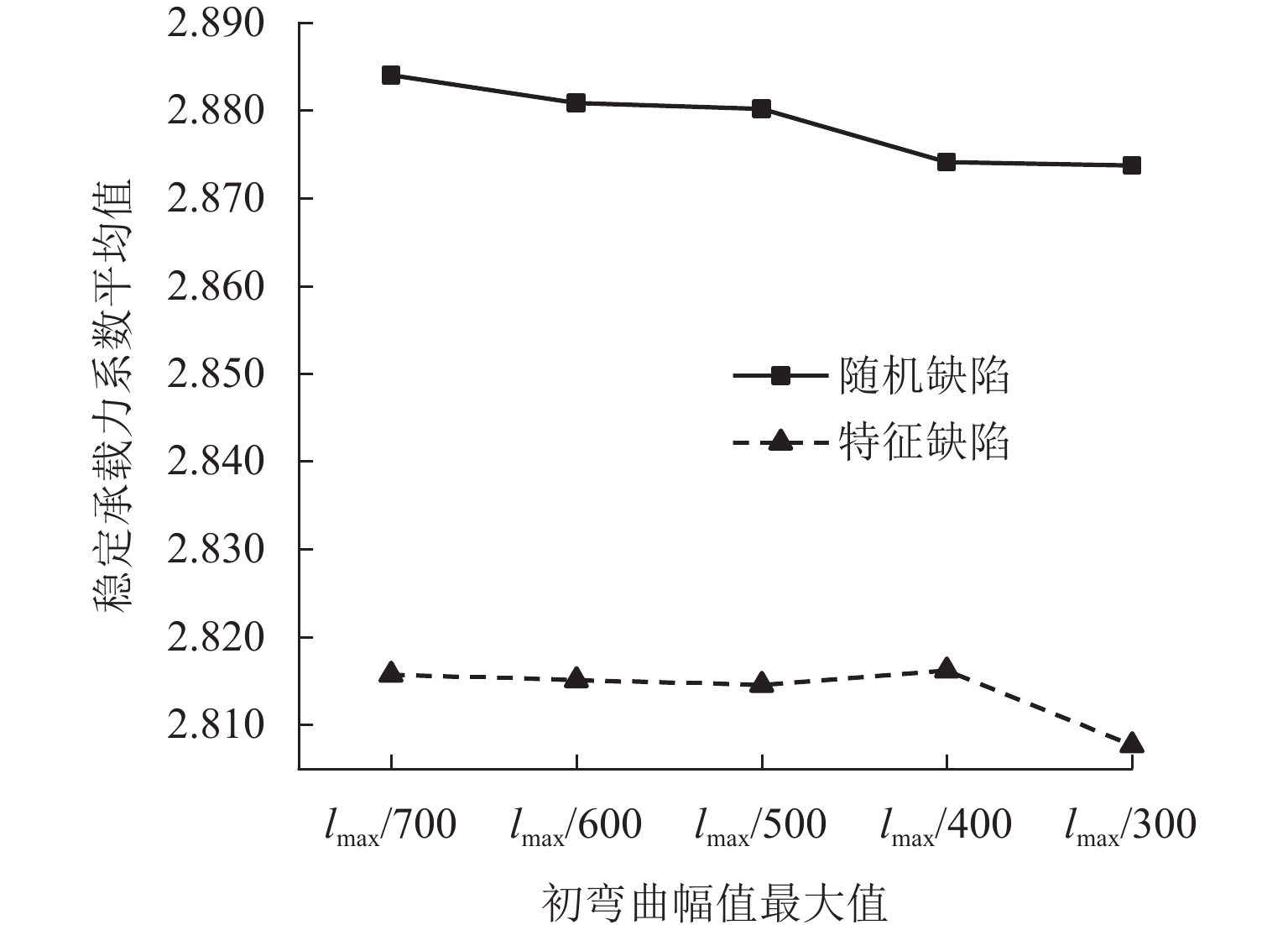
表 4 同时考虑2种缺陷的稳定承载力系数对比Table 4. Comparison of coefficients of stability bearing capacity considering both kinds of imperfections整体缺陷分布模式 初弯曲幅值最大值 Kmin Kmax \mu \sigma 是否满足 χ2 检验 \mu - 3\sigma 随机缺陷 lmax/700 2.845 2.916 2.8840 0.0142 否 lmax/600 2.841 2.914 2.8809 0.0125 是 2.8434 lmax/500 2.843 2.940 2.8802 0.0137 是 2.8391 lmax/400 2.841 2.913 2.8741 0.0164 是 2.8249 lmax/300 2.833 2.912 2.8737 0.0153 是 2.8278 特征缺陷 lmax/700 2.773 2.848 2.8157 0.0129 否 lmax/600 2.770 2.862 2.8151 0.0157 否 lmax/500 2.769 2.859 2.8146 0.0186 否 lmax/400 2.775 2.899 2.8162 0.0198 是 2.7568 lmax/300 2.759 2.878 2.8077 0.0197 否 由表4及图9可见:以随机缺陷为整体缺陷分布模式,引入杆件初弯曲后,随着初弯曲幅值最大值的增大,结构的稳定承载力系数平均值依次减小;当初弯曲幅值最大值取lmax/300时,结构的稳定承载力系数平均值为
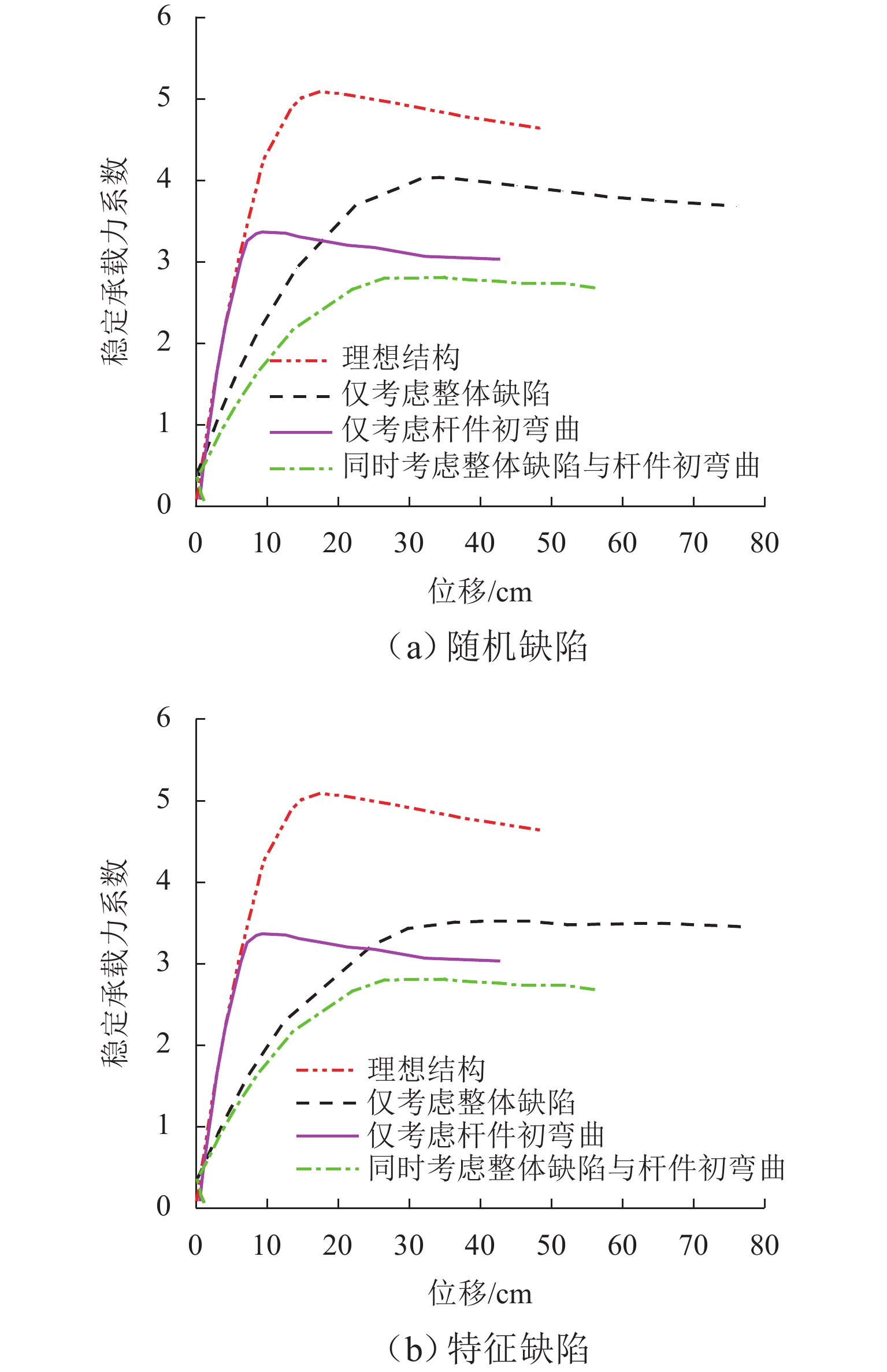
2.8737 ,相比于仅考虑整体缺陷(随机缺陷为4.033)降低了28.75%,降幅明显. 此外,以特征缺陷为整体缺陷分布模式,相比于随机缺陷,引入杆件初弯曲后,结构的稳定承载力系数平均值并非随着初弯曲幅值最大值的增大而呈依次递减的趋势. 当初弯曲幅值最大值取lmax/300时,结构的稳定承载力系数平均值最小(2.8077 ),相比于仅考虑整体缺陷(特征缺陷为3.518)降低了20.19%,降幅同样较大.分别选取理想结构、仅考虑整体缺陷(随机缺陷、特征缺陷)、仅考虑杆件初弯曲及同时考虑整体缺陷(随机缺陷、特征缺陷)和杆件初弯曲的各模型,考察其对应的荷载-位移曲线. 其中,后两者对应的杆件初弯曲形状均取正弦半波、初弯曲幅值最大值均取lmax/300,且其稳定承载力系数分别位于上文分析所得的相应平均值附近. 不同模型对应的荷载-位移曲线对比如图10所示.
由图10可见:仅考虑整体缺陷或杆件初弯曲时,结构的稳定承载力系数分别为4.033(随机缺陷)、3.518(特征缺陷)及3.364,相比于理想结构(5.085),降幅均较大,分别为20.69%、30.82%及33.84%;同时考虑整体缺陷与杆件初弯曲时,结构的稳定承载力系数分别为2.873(随机缺陷)、2.807(特征缺陷),同样相比于理想结构,相应降幅分别为43.50%、44.80%,均小于两者分别引入的降幅之和(54.53%、64.66%). 由此表明,2种缺陷的同时施加,对弦支穹顶结构的稳定承载力存在耦合影响,一定程度上削弱了两者单独引入时的不利影响. 基于此,工程实践中,对类似结构的缺陷稳定性研究时,建议同时考虑2种缺陷的耦合影响.
5. 结 论
1) 相比于理想结构,仅考虑杆件初弯曲时,弦支穹顶结构的稳定承载力系数平均值降幅显著,表明该结构对杆件初弯曲较为敏感. 工程实践中,对类似结构的稳定性分析建议考虑杆件初弯曲的影响.
2) 相比于正弦全波,以正弦半波为初弯曲形状来引入杆件初弯曲,对弦支穹顶结构稳定性能的影响更为不利.
3) 相比于理想结构,同时考虑整体缺陷与杆件初弯曲时,弦支穹顶结构的稳定承载力系数进一步降低,但其降幅小于两者分别引入的降幅之和,表明2种缺陷的同时施加,对弦支穹顶结构的稳定承载力存在耦合影响,一定程度上削弱了两者单独引入时的不利影响. 工程实践中,对类似结构的缺陷稳定性研究时,建议同时考虑2种缺陷的耦合影响.
-
表 1 构件和材料规格
Table 1. Specifications of members and materials
结构部位 构件 材质 规格 上部单层
网壳凯威特
部分径向杆 Q355B ϕ219 × 12 环向杆 Q355B ϕ219 × 12 斜杆 Q355B ϕ203 × 10 联方
部分环向杆 Q355B ϕ203 × 10 斜杆 Q355B ϕ194 × 10 撑杆 Q355B ϕ168 × 8 下部索杆
体系环向索 内圈 平行钢丝
束,1670 级ϕ5 × 61 中圈 平行钢丝
束,1670 级ϕ5 × 91 外圈 平行钢丝
束,1670 级ϕ5 × 139 径向索 平行钢丝
束,1670 级ϕ5 × 55 表 2 仅考虑杆件初弯曲的稳定承载力系数
Table 2. Coefficients of stability bearing capacity considering initial curvature of members only
初弯曲形状 稳定承载力系数 正弦半波 3.361,3.328,3.382,3.390,3.391,3.398,
3.377,3.345,3.338,3.355,3.343,3.367,
3.324,3.401,3.344,3.368,3.371,3.353,
3.375,3.350,3.387,3.379,3.340,3.389,
3.389,3.340,3.320,3.313,3.378,3.401,
3.282,3.378,3.404,3.369,3.370,3.331,
3.444,3.371,3.333,3.342,3.310,3.361,
3.394,3.402,3.338,3.314,3.377,3.348,
3.337,3.359,3.383,3.405,3.358,3.351,
3.360,3.383,3.391,3.329,3.349,3.421,
3.365,3.414,3.346,3.386,3.354,3.339,
3.357,3.393,3.418,3.364,3.339,3.361,
3.364,3.339,3.362,3.345,3.384,3.348,
3.346,3.336,3.356,3.403,3.346,3.350,
3.409,3.375,3.379,3.393,3.377,3.326,
3.355,3.312,3.336,3.397,3.382,3.351,
3.332,3.404,3.397,3.385{K_{\min }} = 3.282 , {K_{\max }} = 3.444 , \mu = 3.3642 , {\sigma } = 0.028\;8 正弦全波 3.424,3.416,3.406,3.416,3.395,3.387,
3.409,3.357,3.400,3.415,3.410,3.392,
3.406,3.395,3.370,3.369,3.390,3.409,
3.380,3.399,3.370,3.400,3.413,3.422,
3.398,3.385,3.405,3.422,3.424,3.423,
3.377,3.401,3.392,3.403,3.421,3.360,
3.408,3.393,3.407,3.411,3.397,3.440,
3.406,3.390,3.392,3.410,3.381,3.391,
3.411,3.397,3.388,3.397,3.409,3.419,
3.388,3.409,3.403,3.366,3.372,3.388,
3.410,3.399,3.367,3.386,3.427,3.412,
3.394,3.401,3.411,3.402,3.383,3.393,
3.400,3.415,3.392,3.427,3.398,3.421,
3.393,3.373,3.387,3.390,3.409,3.395,
3.384,3.395,3.406,3.414,3.401,3.416,
3.343,3.413,3.376,3.406,3.395,3.394,
3.400,3.410,3.387,3.410{K_{\min }} = 3.343 , {K_{\max }} = 3.440 , \mu = 3.3987 , {\sigma } = 0.017\;0 表 3 仅考虑杆件初弯曲的稳定承载力系数对比
Table 3. Comparison of coefficients of stability bearing capacity considering initial curvature of members only
初弯曲幅值最大值 初弯曲形状 Kmin Kmax \mu \sigma 是否满足 χ2 检验 \mu - 3\sigma lmax/700 正弦半波 3.365 3.482 3.4243 0.0178 否 正弦全波 3.424 3.473 3.4453 0.0092 是 3.4177 lmax/600 正弦半波 3.344 3.469 3.4141 0.0216 是 3.3493 正弦全波 3.400 3.465 3.4401 0.0102 是 3.4095 lmax/500 正弦半波 3.356 3.461 3.4065 0.0192 是 3.3489 正弦全波 3.401 3.459 3.4334 0.0109 是 3.4007 lmax/400 正弦半波 3.305 3.476 3.3890 0.0305 是 3.2975 正弦全波 3.369 3.443 3.4215 0.0120 是 3.3855 lmax/300 正弦半波 3.282 3.444 3.3642 0.0288 是 3.2778 正弦全波 3.343 3.440 3.3987 0.0170 是 3.3477 表 4 同时考虑2种缺陷的稳定承载力系数对比
Table 4. Comparison of coefficients of stability bearing capacity considering both kinds of imperfections
整体缺陷分布模式 初弯曲幅值最大值 Kmin Kmax \mu \sigma 是否满足 χ2 检验 \mu - 3\sigma 随机缺陷 lmax/700 2.845 2.916 2.8840 0.0142 否 lmax/600 2.841 2.914 2.8809 0.0125 是 2.8434 lmax/500 2.843 2.940 2.8802 0.0137 是 2.8391 lmax/400 2.841 2.913 2.8741 0.0164 是 2.8249 lmax/300 2.833 2.912 2.8737 0.0153 是 2.8278 特征缺陷 lmax/700 2.773 2.848 2.8157 0.0129 否 lmax/600 2.770 2.862 2.8151 0.0157 否 lmax/500 2.769 2.859 2.8146 0.0186 否 lmax/400 2.775 2.899 2.8162 0.0198 是 2.7568 lmax/300 2.759 2.878 2.8077 0.0197 否 -
[1] KAWAGUCHI M, ABE M, TATEMICHI I. Design, tests and realization of “suspen-dome” system[J]. Journal of the International Association for Shell and Spatial Structures, 1999, 40(3): 179-192. [2] 陈丰,潘睿,王四清. 张家界大成俄罗斯马戏城主馆屋盖结构的稳定性、抗连续倒塌及施工模拟分析[J]. 建筑结构,2020,50(20): 54-58.CHEN Feng, PAN Rui, WANG Siqing. Analysis on the stability, progressive collapse resistance and construction simulation of the roof structure of Dacheng Russian Circus City main pavilion in Zhangjiajie[J]. Building Structure, 2020, 50(20): 54-58. [3] 李璐. 张弦结构在景德镇游泳馆中的应用[J]. 建筑结构,2021,51(增2): 335-339.LI Lu. Application of string structure in Jingdezhen swimming center[J]. Building Structure, 2021, 51(S2): 335-339. [4] 葛家琪,张国军,王树,等. 2008奥运会羽毛球馆弦支穹顶结构整体稳定性能分析研究[J]. 建筑结构学报,2007,28(6): 22-30,44. doi: 10.3321/j.issn:1000-6869.2007.06.003GE Jiaqi, ZHANG Guojun, WANG Shu, et al. The overall stability analysis of the suspend-dome structure system of the badminton gymnasium for 2008 Olympic Games[J]. Journal of Building Structures, 2007, 28(6): 22-30,44. doi: 10.3321/j.issn:1000-6869.2007.06.003 [5] 刘静,赵若旭,陈宗学,等. 河北北方学院体育馆弦支穹顶屋盖稳定性能分析[J]. 建筑结构,2020,50(5): 82-87.LIU Jing, ZHAO Ruoxu, CHEN Zongxue, et al. Stability analysis on the suspend-dome structure roof of Hebei North University gymnasium[J]. Building Structure, 2020, 50(5): 82-87. [6] 陈前吉. 800 m凯威特巨型网格弦支穹顶静力及稳定性研究[D]. 哈尔滨:哈尔滨工业大学,2018. [7] 姜正荣,王仕统,石开荣,等. 厚街体育馆大跨度椭圆抛物面弦支穹顶结构的非线性屈曲分析[J]. 土木工程学报,2013,46(9): 21-28.JIANG Zhengrong, WANG Shitong, SHI Kairong, et al. Nonlinear buckling analysis of long-span elliptic paraboloid suspended dome structure for Houjie Gymnasium[J]. China Civil Engineering Journal, 2013, 46(9): 21-28. [8] LIU X C, ZHAN X X, ZHANG A L, et al. Random imperfection method for stability analysis of a suspended dome[J]. International Journal of Steel Structures, 2017, 17(1): 91-103. doi: 10.1007/s13296-015-1234-3 [9] 于敬海,胡相宜,陈彦,等. 弦支穹顶初始几何缺陷分布及稳定安全系数取值研究[J]. 空间结构,2017,23(3): 3-10,20.YU Jinghai, HU Xiangyi, CHEN Yan, et al. Research on initial geometric imperfection distribution and stability safety factor of suspen-dome[J]. Spatial Structures, 2017, 23(3): 3-10,20. [10] 王琼,邓华,黄莉. 杆件初弯曲对弦支穹顶静动力承载力的影响[J]. 空间结构,2013,19(4): 18-24,33. doi: 10.3969/j.issn.1006-6578.2013.04.003WANG Qiong, DENG Hua, HUANG Li. Effect of member’s initial curvature on the static and dynamic bearing capacity of suspended dome[J]. Spatial Structures, 2013, 19(4): 18-24,33. doi: 10.3969/j.issn.1006-6578.2013.04.003 [11] DING Y, ZHANG T L. Research on influence of member initial curvature on stability of single-layer spherical reticulated domes[J]. Advanced Steel Construction, 2019, 15(1): 9-15. [12] ZHAO Z W, LIU H Q, LIANG B, et al. Influence of random geometrical imperfection on the stability of single-layer reticulated domes with semi-rigid connection[J]. Advanced Steel Construction, 2019, 15(1): 93-99. [13] 闫翔宇,巩昊,陈志华,等. H型钢弦支穹顶结构弹塑性稳定性分析[J]. 空间结构,2022,28(3): 39-48.YAN Xiangyu, GONG Hao, CHEN Zhihua, et al. Elastic-plastic stability analysis of H-beam suspendome[J]. Spatial Structures, 2022, 28(3): 39-48. [14] 严佳川. 考虑杆件初弯曲的网壳结构耦合失稳机理研究[D]. 哈尔滨:哈尔滨工业大学,2012. [15] 陈惠发,ATSUTA T. 梁-柱分析与设计[M]. 北京:人民交通出版社,1997. [16] 邱俊明. 弦支穹顶结构的预应力多目标优化及静力稳定性分析[D]. 广州:华南理工大学,2022. [17] 李冬村. 凯威特-联方型单层网壳结构的静力稳定性及局部破坏敏感性分析[D]. 广州:华南理工大学,2019. [18] 刘红亮. 大跨度弦支穹顶结构的缺陷敏感区域及动力稳定性研究[D]. 广州:华南理工大学,2015. [19] 中华人民共和国住房和城乡建设部. 空间网格结构技术规程:JGJ 7—2010[S]. 北京:中国建筑工业出版社,2011. -






 下载:
下载:










 下载:
下载: