Comparison of Stress-Dilatancy Rules and Research on Stress-Dilatancy Rule for Rocks
-
摘要:
为研究典型应力-剪胀关系对常见岩土材料力学响应的预测效果,构建适合岩石材料的应力-剪胀关系,提升本构模型的准确性,本文结合试验数据对典型应力-剪胀关系展开对比分析,并构建适用于岩石的应力剪胀关系模型. 首先,基于热动力学框架和能量守恒方程,梳理出3种典型的应力-剪胀关系模型,并将多种岩土材料的应力-剪胀数据与典型应力-剪胀关系进行对比;随后,以Rowe剪胀关系模型为基本框架,考虑多种因素影响,提出适用于岩石的改进Rowe应力-剪胀关系模型,分析了其对试验数据的拟合效果,并对比所提模型和变剪胀角模型对加载过程剪胀角演化的模拟效果;最后,将修正Rowe剪胀关系与修正剑桥模型耦合,与经典的修正剑桥模型的模拟结果和试验数据进行对比校验. 研究结果表明:由于黏聚力的影响,基于纯摩擦假设推导的经典应力-剪胀关系无法准确描述含黏聚力岩土材料的应力-剪胀响应;修正Rowe剪胀关系模型可以有效反映岩石的应力-剪胀响应,并能模拟数据中的“回旋勾”现象;本文提出的应力-剪胀关系模型不仅形式简洁,且参数数量较变剪胀角模型少;应用所提修正Rowe剪胀关系模型可以提升本构模型在变形预测方面的准确性.
Abstract:In order to evaluate the prediction accuracy of typical stress-dilatancy rules on the mechanical response of common geomaterials, propose a stress-dilatancy rule suitable for rocks, and improve the accuracy of constitutive models, typical stress-dilatancy rules were compared with experimental data to propose a stress-dilatancy model suitable for rocks. Firstly, based on the thermodynamic framework and the energy conservation equation, three typical stress-dilatancy models were sorted out, and the stress-dilatancy data of various geomaterials were compared with those typical stress-dilatancy rules. Then, by taking the Rowe dilatancy model as the basic framework and considering the influence of various factors, an improved Rowe stress-dilatancy model suitable for rocks was proposed, and its fintness on the experimental data was analyzed. The fitness of the proposed model and the variable dilatancy angle model on the evolution of dilatancy angle during loading was compared. Finally, the modified Rowe dilatancy rule was coupled with the modified Cam-clay model, and the simulation results and test data of the classical modified Cam-clay model were compared and verified. The results show that the classical stress-dilatancy rule based on the pure friction hypothesis cannot accurately describe the stress-dilatancy response of geomaterials with cohesion due to the influence of cohesion. The modified Rowe dilatancy model can effectively reflect the stress-dilatancy response of rock and simulate the “turning hook” phenomenon in the data. Furthermore, the proposed stress-dilatancy model is not only simple in form but also has fewer parameters than the mobilizing dilatant angle models. The proposed modified Rowe dilatancy model can improve the accuracy of the constitutive model in deformation prediction.
-
Key words:
- stress-dilatancy rule /
- rocks /
- cohesive force /
- dilatancy angle /
- models
-
应力-剪胀关系模型将应力状态(应力比)和剪胀系数联系起来,反映了岩土材料的剪胀性,是岩土材料本构模型的关键要素之一[1-5]. 建立合适的应力-剪胀关系,以准确描述岩土材料在剪切过程中的体积变化情况,进而在弹-塑性本构模型中更准确地反映岩土材料的力学行为,并为工程设计和优化提供可靠的理论依据,是岩土材料本构理论的重要研究内容.
Rowe[6]通过对两相互接触块体的相互摩擦进行力学建模,引入摩尔-库伦准则,推导了经典的Rowe应力-剪胀关系模型. 在Rowe模型基础上,有学者考虑块体接触面的相互黏结效应(即黏聚力),提出了考虑黏聚力效应的Rowe应力-剪胀关系表达式[7]. 在剑桥模型的构建中,Roscoe等[8]借助Taylor[9]的方法,基于热动力学框架和能量守恒,提出了Cam-clay应力-剪胀关系. 随后,在对剑桥模型的改进过程中,相关学者又提出了修正Cam-clay[10]应力-剪胀关系模型. Rowe应力-剪胀关系模型、Cam-clay和修正Cam-clay应力剪胀关系模型等由于形式简洁、物理意义突出,被广泛应用于岩土力学计算. 此外,Tsegaye等[11-12]借助能量守恒定律并采用修正后的应力-剪胀关系假说,分别构建了适用于岩土材料和岩体的应力-剪胀关系框架. 由于应力-剪胀关系形式简洁、物理意义清晰等特点,Jefferies等[13-15]学者在Cam-clay应力-剪胀关系基础上进行改进,形成了考虑不同力学特性的应力-剪胀关系模型.
随着岩土力学试验手段的发展和相关研究的深入,研究人员逐渐发现,经典的应力-剪胀关系模型无法有效地描述部分岩土材料在颗粒破碎等情况下的剪胀行为[16]. 在这一背景下,国内外研究人员建立了考虑颗粒破碎的堆石料、钙质砂等[17]岩土材料的应力-剪胀关系模型. 由于很多应力-剪胀关系都是对剑桥模型[8]、修正剑桥模型[10]和Rowe[6]这3种典型应力-剪胀关系的修正,一定程度上继承了这些模型的单值性,无法有效反映应力-剪胀试验数据中的“回旋勾”等特征[17-18]. 此外,对岩石而言,目前大多数岩石剪胀特性的研究是以岩石剪胀角[18-21]为研究对象,所构建的部分模型所需参数太多且偏向经验模型,不利于参数标定和工程应用. 此外,部分研究人员[22-25]在总结梳理大量试验数据的基础上,对岩石的应力-剪胀响应特征进行分析,并以经验化的方程反映岩石的应力-剪胀特性,虽有助于提升模拟效果,但有可能掩盖其物理意义. 因此,亟需构建适用于岩石的应力-剪胀关系,以准确描述岩石在加、卸载过程中发生的扩容或剪胀现象,并建立更准确可靠的岩石本构模型.
本文首先基于热力学框架和能量守恒方程,梳理了3种典型应力-剪胀关系[8-10]. 为发掘黏聚力的存在和丧失对岩石应力-剪胀关系的影响特征,将3种典型应力-剪胀关系[8-10]和砂土[26]/堆填料[17,27]、水泥/生物水泥[28-29]加固砂土、软岩[25]/硬岩[30]等岩土材料的试验数据进行对比分析. 进一步,提出适用于岩石的改进Rowe应力-剪胀关系模型,该模型考虑了黏聚力和塑性剪切应变累积等因素的影响. 随后,本文将所提应力-剪胀关系与软岩/硬岩试验数据进行对比,并与变剪胀角模型预测的剪胀角演化进行对比,对本文所提应力-剪胀关系的有效性进行校验. 最后,基于本文将所提出的改进Rowe应力-剪胀关系,结合修正剑桥模型,推导了塑性应变增量的表达式,并对比试验数据和经典修正剑桥模型的模拟结果,对本文所提应力-剪胀关系进行进一步检验.
1. 典型应力-剪胀关系
20世纪60年代以来,随着一大批岩土材料弹-塑性本构模型的构建,涌现出多种不同的应力-剪胀关系模型. 构建的应力-剪胀关系模型大多根据岩土材料的特性,采用了不同的假设条件和简化方式,故形成了不同的表达形式.
d=f(η,M,α,⋯), (1) 式中:d=δεpv/δεpγ,为剪胀系数,即塑性体积应变增量δεpv和塑性剪切应变增量δεpγ的比值,d<0体积膨胀,d>0体积收缩;η=q/p,为应力比,p为净水压力,q为等效剪切应力;M为临界状态应力比;α为应力-剪胀关系其他参数,如弹塑性本构中的内变量.
随着本构理论的进一步发展,基于热动力学理论框架和能量守恒等基本物理准则[26]可用于应力-剪胀关系的导出和统一描述. 在等温假设和满足热力学第二定律条件下,能量守恒方程为
We=Φ+Ψ, (2) 式中:We为外力做功,Φ为能量消耗,Ψ为Helmholtz自由能.
通过假设上述3项的不同形式,并在式(2)两边除以p得到应力比η,再利用剪胀系数的定义可以导出典型的剑桥模型[8]、修正剑桥模型[10]和Rowe[6]应力-剪胀关系. 对应的式(2)中的各项形式如表1所示,pc为临界状态下的平均应力.
2. 典型应力-剪胀关系对比研究
本节将对比不同岩土材料的应力-剪胀试验数据和上述应力-剪胀关系的预测效果. 通过对无黏性材料、人工黏性材料、岩石材料的应力-剪胀响应和理论模型的比较,分析黏聚力的存在和丧失对包括岩石等黏性材料的应力-剪胀关系的影响规律,并为适用于岩石的应力-剪胀关系构建提供帮助.
2.1 砂土/大坝堆填料的应力-剪胀关系对比
图1为砂土/大坝堆填料的应力-剪胀关系对比. 从图1可知:对于Nevada砂[27],3种应力-剪胀关系表达式均可较好地反映试验数据中的趋势,并能给出较为合理的剪胀系数d预测值;对紫坪铺填料[28],修正剑桥模型应力剪胀关系模型的预测与试验数据大致符合,但未能有效反映峰值应力比附近的剪胀系数,而Rowe模型则能更好地贴近峰值区的应力剪胀系数.
通过对比可见:由于砂土和大坝堆填料均属于无黏性材料,两者的应力-剪胀响应接近“纯摩擦”状态,试验数据的大体趋势与3种典型模型较为接近. 由于相关函数的单值性,3种典型的应力-剪胀关系均不能模拟试验数据中的“回旋勾”[17-18].
2.2 水泥/生物水泥加固砂土的应力-剪胀关系对比
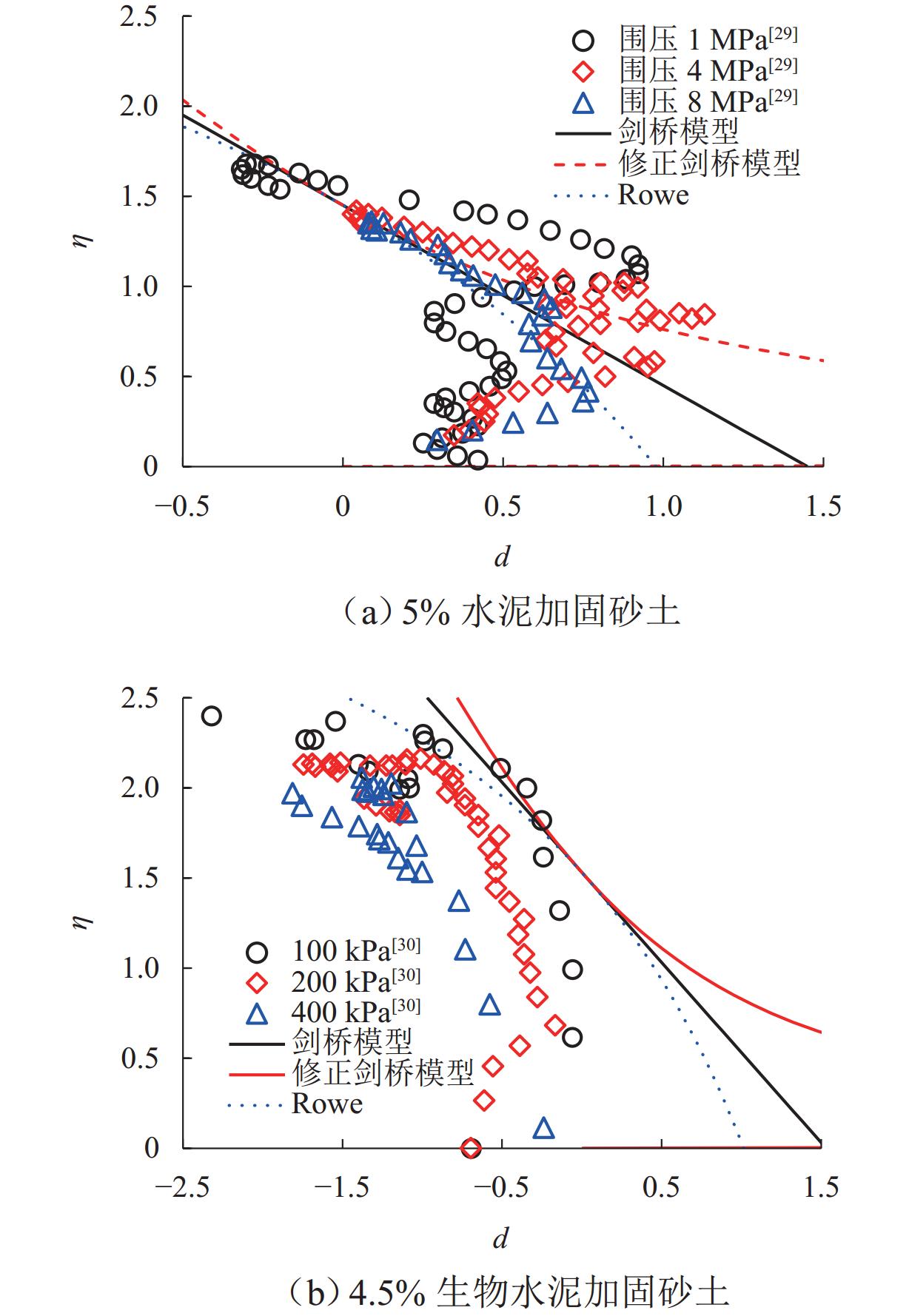
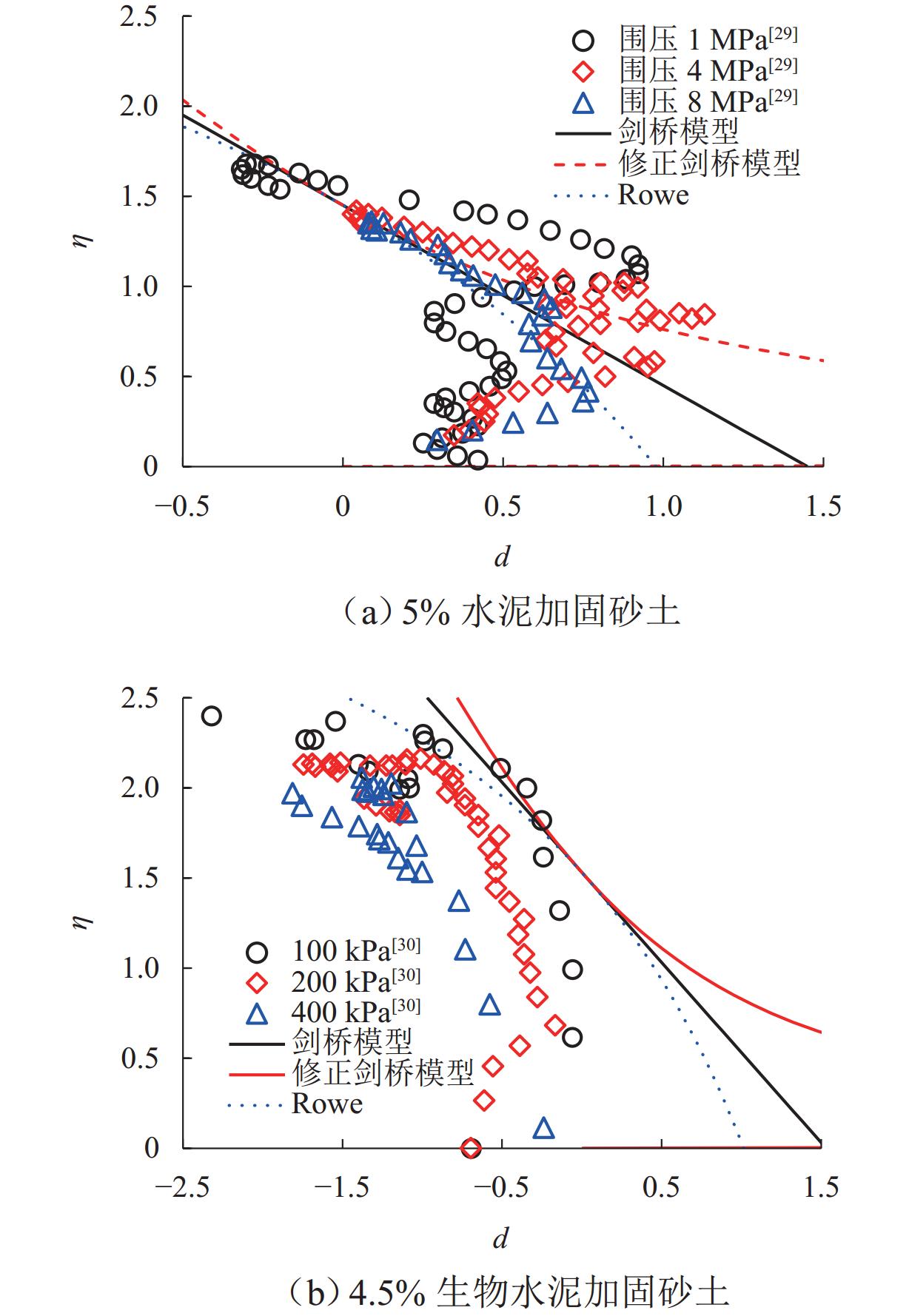
图2给出了5%水泥加固砂土[29]和平均占比为4.5%生物水泥加固砂土[30]在不同围压下开展常规三轴压缩试验所得的应力-剪胀响应曲线. 从图中可以发现:相比图1中的砂土和堆填料,由于水泥加固和生物水泥加固给砂土增加了“黏结”,进而增强了相关试样的黏聚力,试验数据中的“回旋勾”现象[17-18]更为明显,且在峰值应力比附近存在一定的“平台”区,在生物水泥加固砂样品中更为显著;相较于剑桥模型和修正剑桥模型应力-剪胀关系,Rowe应力剪胀关系能够较好地反映低围压试验数据的大致趋势和峰值应力比附近的剪胀系数;剑桥模型和修正剑桥模型应力-剪胀关系在峰值应力比附近计算所得的剪胀系数更小,无法有效预测带黏聚力岩土材料如水泥加固砂土等的剪胀性.
从图中还可以看出:由于水泥或生物水泥给砂土带来了“附加黏聚力”,使得图2中的应力-剪胀关系与图1中无黏性材料的应力-剪胀响应相异,试验数据不仅在趋势上偏离3种典型模型的预测,在峰值应力比附近还出现了一个类似“平台”的区域.
2.3 软岩/硬岩的应力-剪胀关系对比
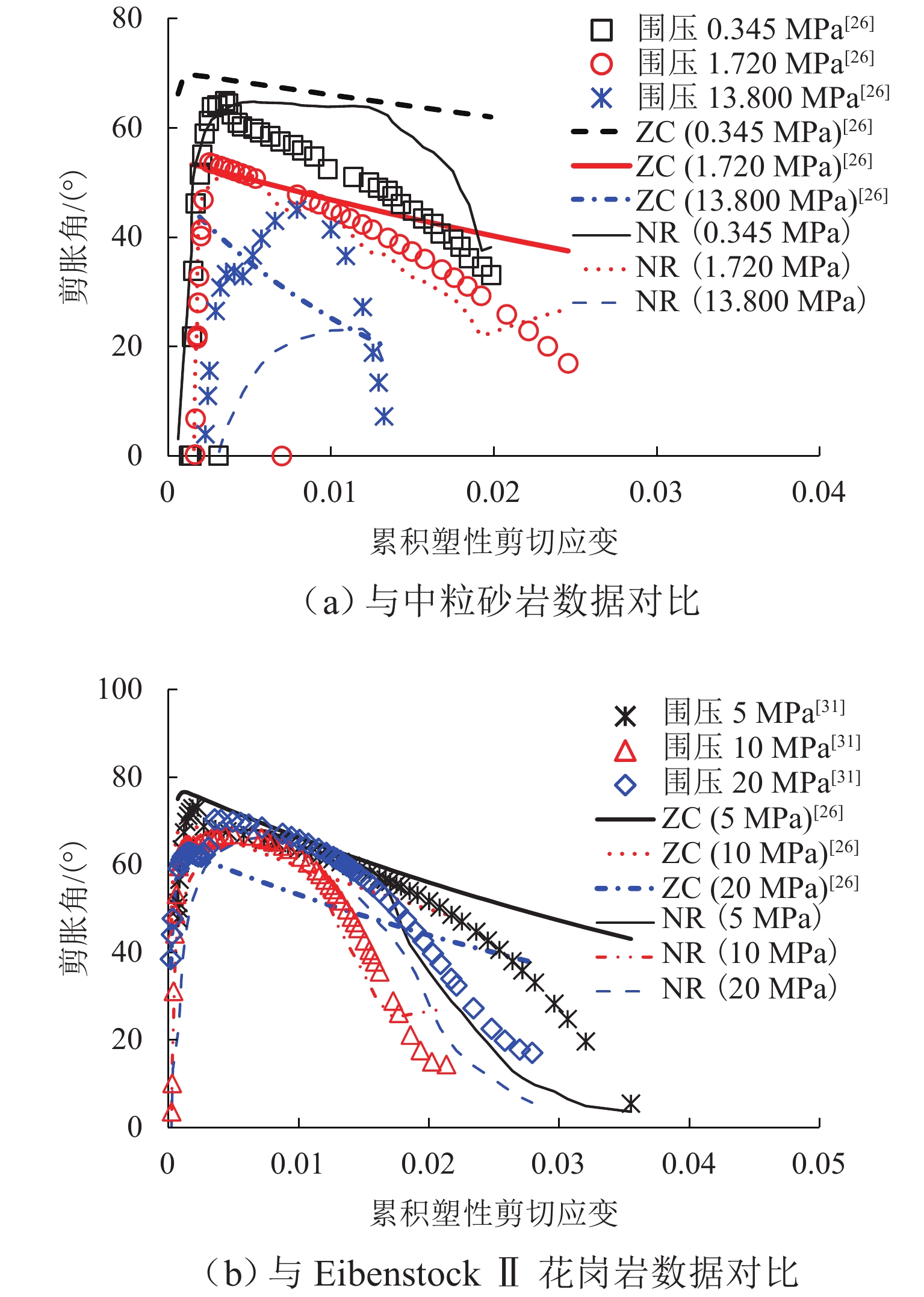
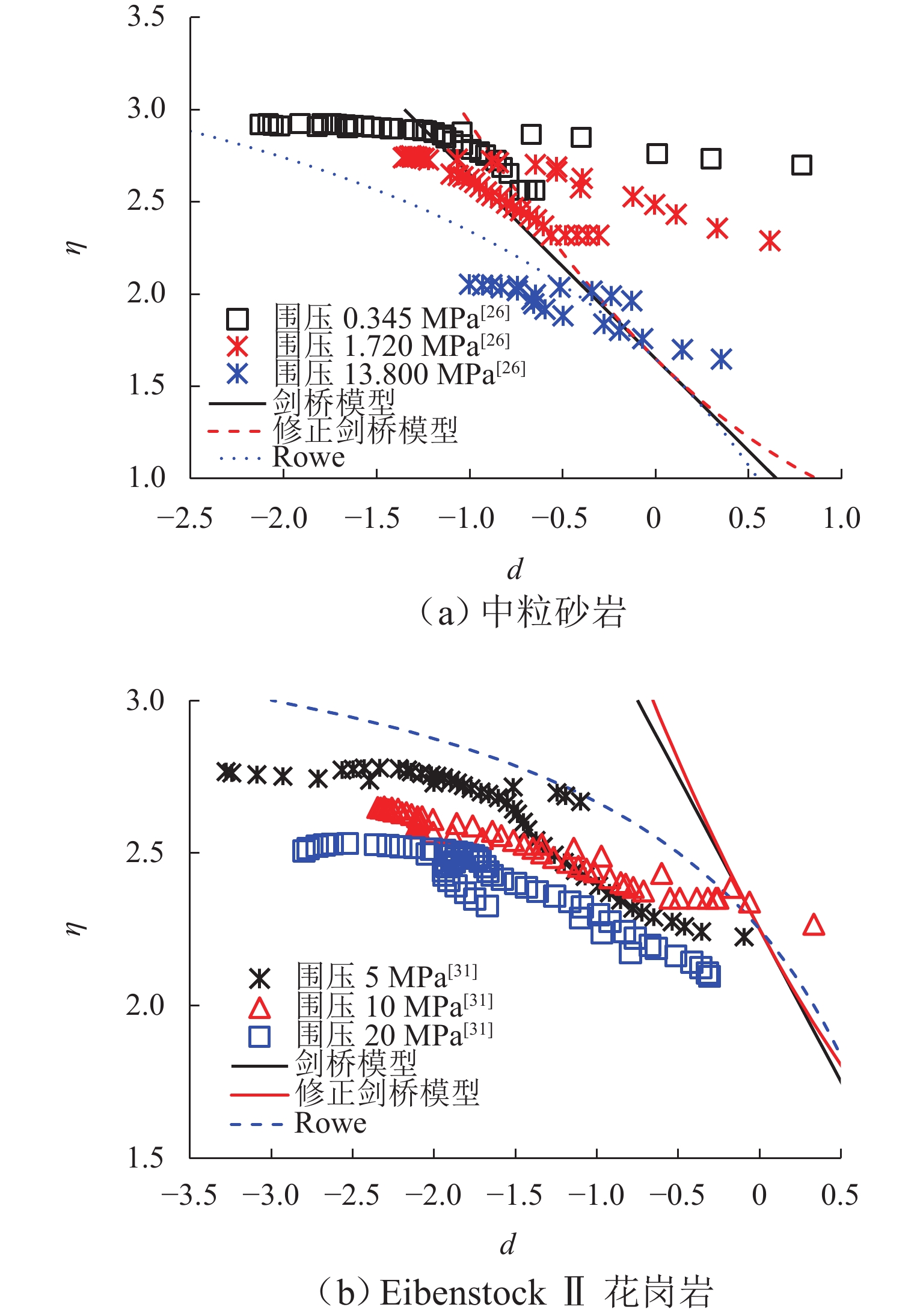
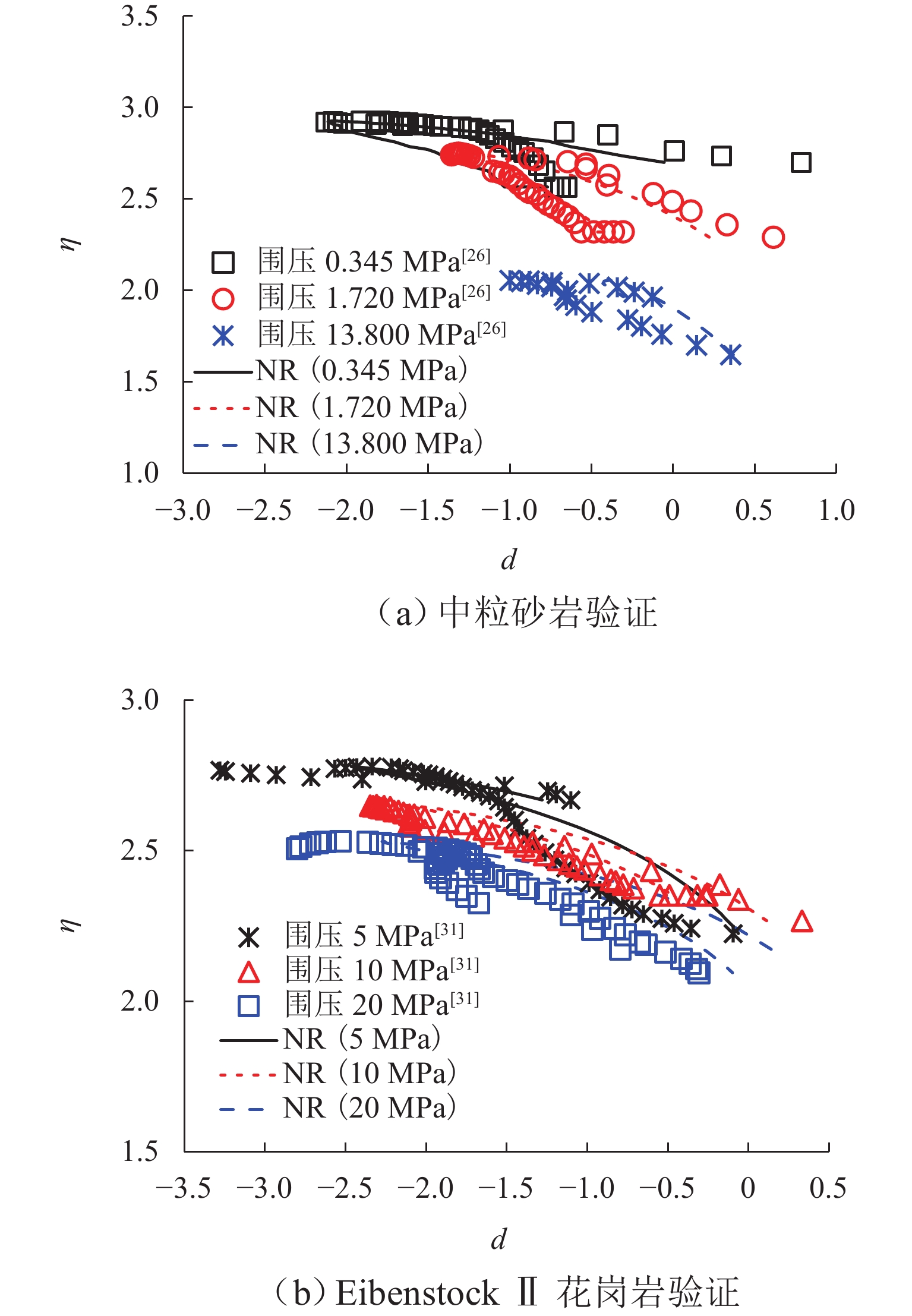
本节选取了典型软岩(中粒砂岩[26])和典型硬岩(Eibenstock Ⅱ 花岗岩[31-32])在不同围压下的常规三轴压缩试验应力-剪胀数据,并与3种典型的应力-剪胀关系模型预测进行比较,如图3.
从图3中可以发现:在3种应力-剪胀关系模型中,Rowe应力-剪胀关系模型能较好地反映部分围压下的试验趋势,如中粒砂岩在13.8 MPa和Eibenstock Ⅱ花岗岩在5.0 MPa下的数据;剑桥模型和修正剑桥模型应力-剪胀关系模型均无法有效反映各围压下的应力-剪胀关系,所预测的峰值应力附近的剪胀系数远小于试验值;虽然Rowe应力-剪胀关系模型也无法预测全部围压下的试验数据,但对于峰值应力比附近的剪胀系数,其预测值比其余2种模型更接近试验值. 此外,3种典型应力-剪胀关系模型均无法反映试验数据中的“回旋勾”[17-18];与具有较弱黏聚力的水泥加固砂的应力-剪胀关系相比,岩石由于具有更强的黏聚力,且黏聚力在加载过程中逐渐消失,从而释放能量,相比图2,峰值应力比附近的“平台”区域更明显. 因此,在构建适用于岩石的应力-剪胀关系过程中,必须考虑黏聚力的影响.
3. 改进Rowe应力-剪胀关系
3.1 含黏聚力材料的应力-剪胀特性
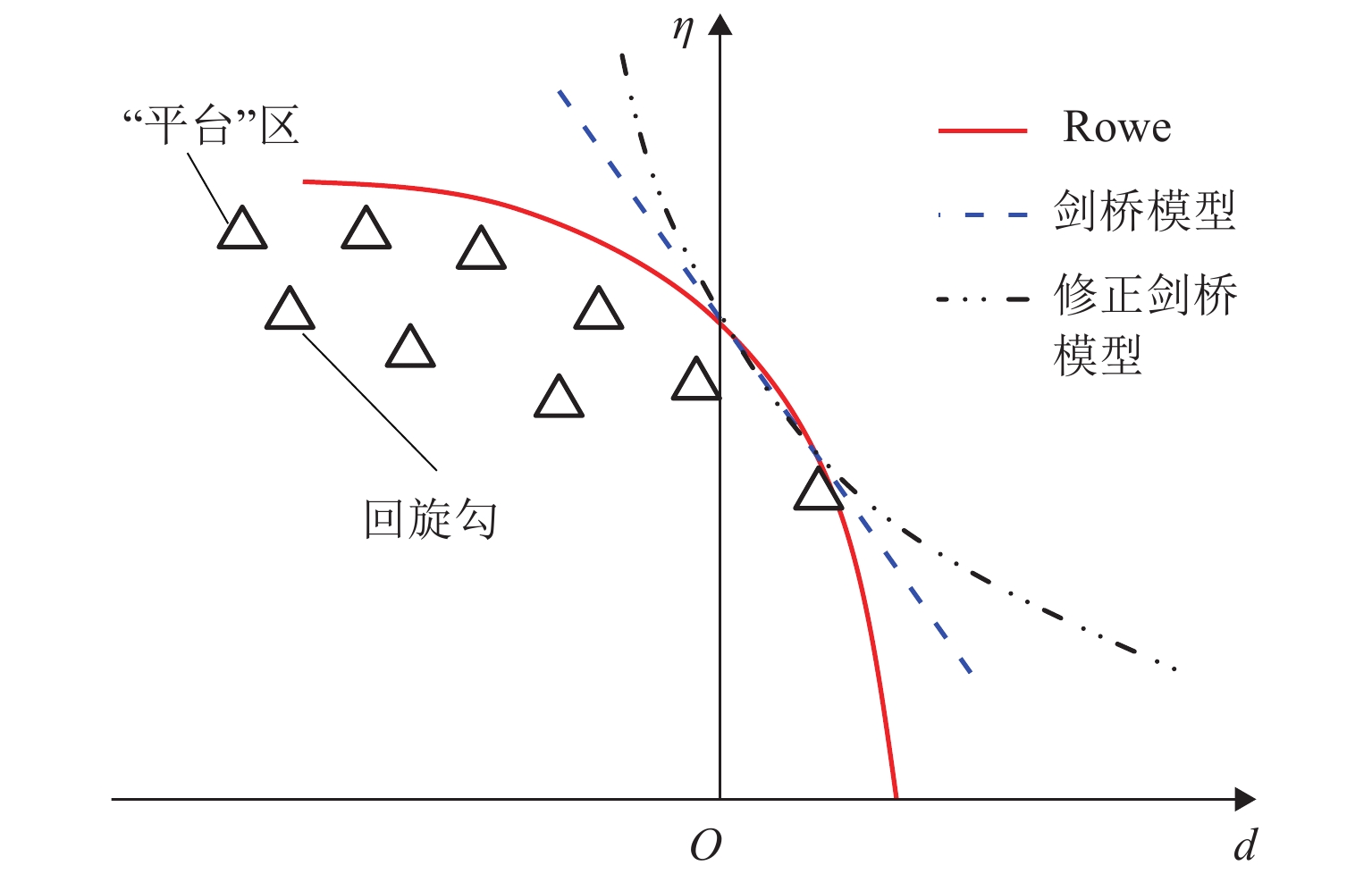
黏聚材料如岩石和水泥加固砂土的应力-剪胀响应主要特征如图4所示. 从图4中可见:由于黏聚力的存在,在加载初始阶段到峰值应力比附近,部分加载能量被用于破坏黏聚力,故无法基于纯摩擦假设描述其应力-剪胀关系,实际响应与剑桥模型、修正剑桥模型的应力-剪胀关系预测值有较大偏离;在加载到峰值应力比附近,由于大量裂纹扩展,岩石试样产生较大的体积变形,故在峰值应力比区域有一个体积显著膨胀的“平台”区;在黏聚力完全破坏的加载后期,材料接近纯摩擦状态,试样的应力-剪胀响应逐渐往纯摩擦状态靠近,其体积变形随着试样的软化而逐渐收敛. 结合图2、3可以发现:虽然Rowe应力剪胀关系可以较好地描述峰值应力比附近的“平台”区,由于其自身的单值性,无法反映“回旋勾”,也无法反映围压增加造成的应力-剪胀曲线整体下移.
3.2 改进Rowe应力-剪胀关系
通过对3种典型的应力-剪胀关系模型预测与试验数据的对比分析可以发现:相比剑桥模型和修正剑桥模型应力-剪胀关系模型,Rowe应力-剪胀关系模型能更好地预测试验现象. 为克服Rowe应力-剪胀关系模型的单值性,本节将考虑围压影响,引入累计塑性应变重构式(2)中的外力做功、能量消耗和Helmholtz自由能项,进而构建可以有效反映试验数据的改进Rowe应力-剪胀关系模型.
为考虑围压效应,引进围压相关的系数K,将Helmholtz自由能假设为式(3)的形式.
Ψ=K9(pδεpv−pδεpγ). (3) 同时,引进累计塑性应变εpγ,将能量消耗方程形式调整为
Φ=[1+Eexp(−bεpγ)]Mpδεpγ+2q−3p9[1+Eexp(−bεpγ)]Mδεpγ, (4) 式中:E、b为与塑性剪切应变有关的参数,Eexp(−bεpγ)为黏聚力破坏过程中消耗的能量.
假设外力功率We为pδεpv+qδεpγ,将式(3)、(4)代入式(2),在两边除以p和δεpγ,并利用d=δεpv/δεpγ可以得到修正Rowe应力-剪胀关系为
d=9(M(εpγ)−η)−K9+3M(εpγ)−2M(εpγ)η−K, (5) 式中:M(εpγ)=(1+Eexp(−bεpγ))M;K=σc/(ξ+ωσc),ξ和ω均为模型参数,表征不同围压σc下参数K取值.
3.3 改进Rowe应力-剪胀关系特性
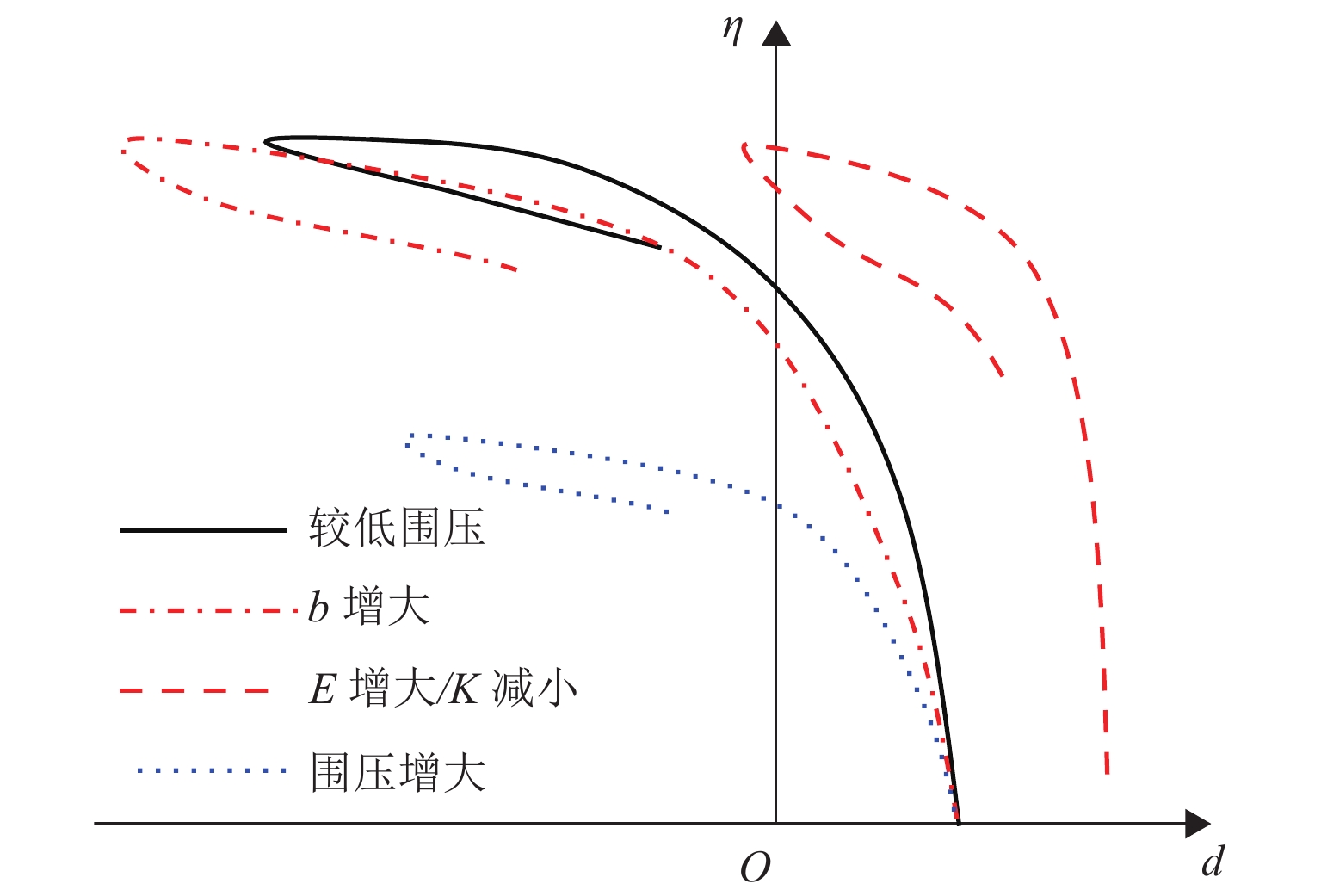
结合上述关于修正Rowe应力-剪胀关系方程,对其影响较大的3个参数(E、b、K)以及围压对预测应力-剪胀关系的影响如图5. 由图5可见:以黑色实线为参考,随着围压增加,应力-剪胀曲线沿着应力比η轴向下平移,在水平方向整体向右缩小,表明随着围压增加,岩石材料的体积膨胀幅度逐渐减小;随着参数E和ω的增大,如图5中虚线所示,预测的应力-剪胀曲线整体向右平移,并出现一个水平方向的明显收缩;参数b增大时,如点划线所示,曲线在水平方向上向左拉伸,产生更大的体积膨胀.
4. 模型验证与讨论
4.1 模型验证
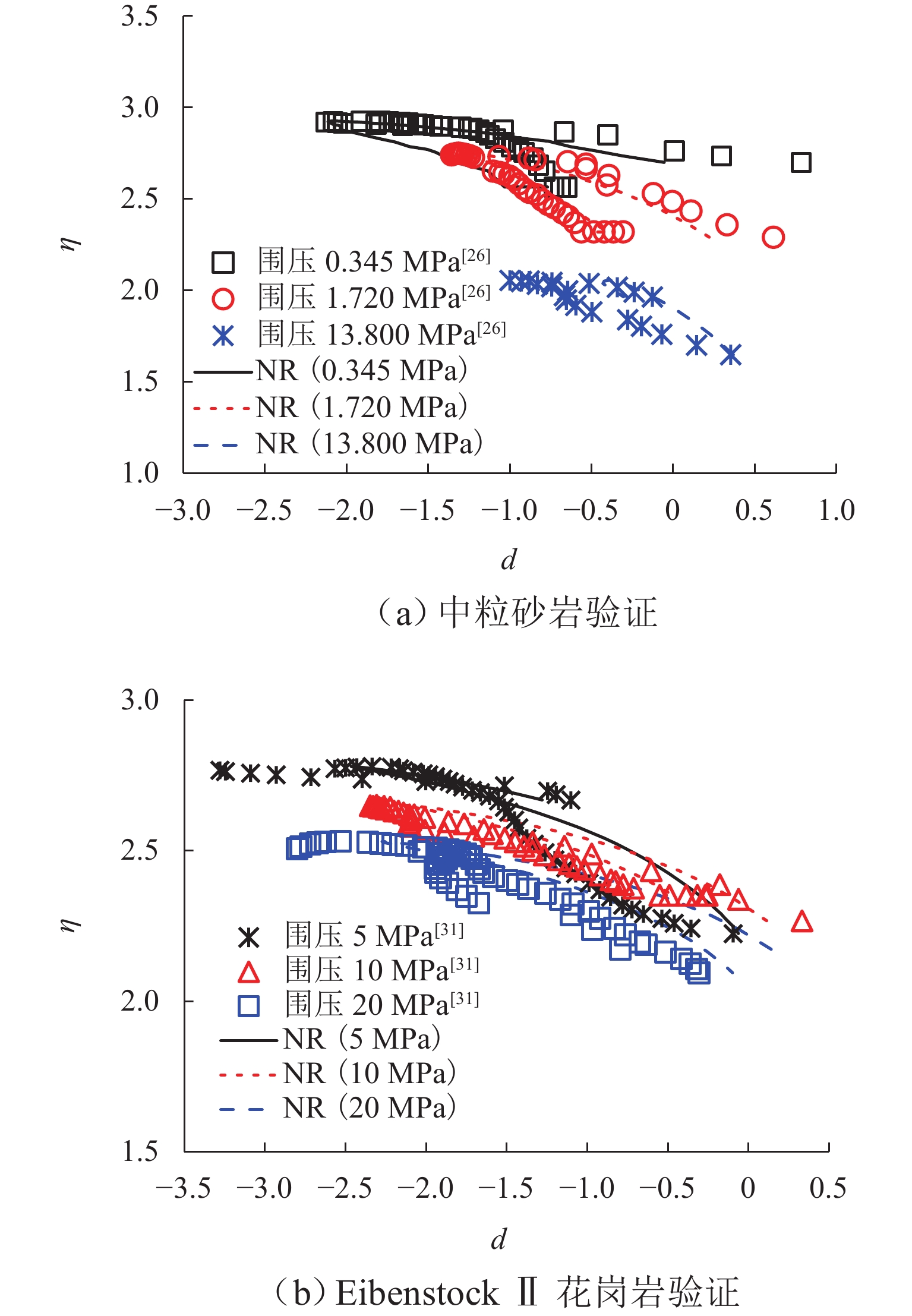
采用图3所示的2种岩石试验数据对式(5)中的改进Rowe应力-剪胀关系模型进行校验,2种岩石对应的4个参数取值如表2所示,所得的应力-剪胀预测曲线及与不同围压下试验数据的对比如图6所示,NR为本文所提的改进Rowe应力-剪关系模型.
表 2 改进Rowe准则参数Table 2. Parameters of modified Rowe rule岩石 E b ξ ω 中粒砂岩 30 0.30 6.00 0.10 Eibenstock Ⅱ花岗岩 50 0.10 4.80 0.20 从图6中可见:NR可以有效地反映软岩和硬岩在不同围压下的应力-剪胀特性. 将剪切应变、围压、黏聚力等因素纳入改进Rowe准则中,本文所提的改进Rowe准则可以反映试验数据中存在的“回旋勾”现象,并能对峰值应力比及附近的剪胀系数数值给出较好的预测. 由此可见,本文所提出的应力-剪胀关系能够很好地反映岩石的应力-剪胀特性.
4.2 与变剪胀角模型的对比
为进一步研究本文所提出的应力-剪胀关系模型对剪胀角ϑ的预测效果,利用剪胀系数与剪胀角的关系(d=tanϑ)将图6中的试验数据和本文的预测结果换算为剪胀角,剪胀角随塑性剪切应变的变化及与Zhao和Cai所提出的变剪胀角理论模型(ZC)[25]的对比如图7.
从图7中可以发现:相比Zhao和Cai的模型,本文所提出的改进Rowe关系模型能更好地反映试验数据中剪胀角的变化;在Zhao和Cai的模型中,所涉及到的3个模型参数均需随着围压改变而变化,实际上共需9个参数;本文所提准则中仅参数B需要随围压变化而变化,共需定义4个参数. 可见,本文所提出的改进Rowe应力-剪胀关系模型在保证模型简单的前提下,能更好地反映岩石的剪胀性,具有独特的便捷性.
4.3 修正Rowe应力-剪胀关系的应用
在修正剑桥模型(MCC)中,对式(6)的屈服面进行求导,可得屈服面上的方向向量n,并对其进行标准化,如(7).
f=q2−M2[p(pa−p)], (6) 式中: M=qc/pc,qc为临界状态下的剪切应力;pa为屈服面的大小.
n=[npnq]=[∂f/∂p√(∂f/∂p)2+(∂f/∂q)2∂f/∂q√(∂f/∂p)2+(∂f/∂q)2], (7) 式中:np为屈服面的方向向量在p轴的分量,nq为在q轴的分量.
在获得应力-剪胀关系的表达式后,可得塑性流动方向向量m为
m=[mpmq]=[d√d2+11√d2+1], (8) 式中:mp为塑性流动方向向量在p轴的分量,mq为在q轴的分量.
结合屈服面上的塑性一致条件[9],可得塑性应力-应变增量表达式为
[δεpvδεpγ]=1H[npmpnqmpnpmqnqmq][δpδq], (9) 式中:H为塑性模量,如式(10).
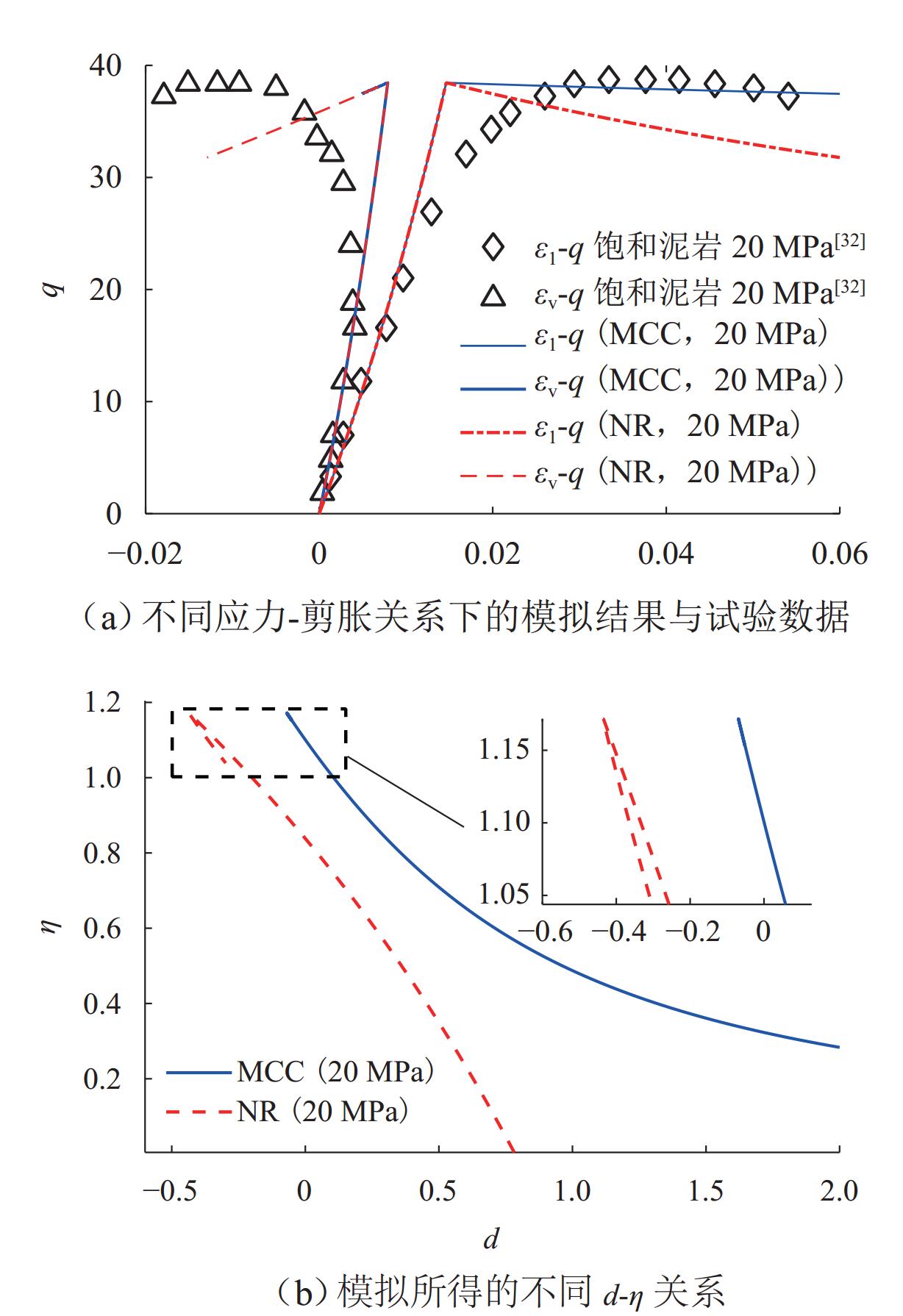
H=−∂f∂paδpaδεpvmp√(∂f/∂p)2+(∂f/∂q)2. (10) 结合文献[10]中弹性部分的描述和经典修正剑桥模型应力-剪胀关系,可以对常规三轴下饱和泥岩的应力-应变响应进行模拟,计算参数如表3所示,根据修正剪切模型的有关规定,表3中:v0为初始体积比,μ为泊松比,κ为压缩曲线弹性段的斜率,λ为弹塑性段的斜率. 模拟结果和所得的应力-剪胀关系如图8所示,图中:εv为体积应变,ε1为轴向应变.
表 3 模拟所用参数Table 3. Parameters for simulation参数 取值 μ 0.23 κ 0.015 λ 0.15 M 1.10 pc0/MPa 70 v0 1.258 ξ 4 ω 0.15 E 0.05 b 20 从图8中可以发现:相较修正剑桥模型中的应力-剪胀关系,采用如式(4)所示的修正Rowe关系所得的应力-应变关系,更能反映试验数据的趋势. 修正剑桥模型的应力-剪胀关系无法有效描述岩石材料的应力-剪胀响应,导致其低估了泥岩加载过程中的体积增加,无法有效反映其侧向变形或体积变形. 而采用本文所提的改进Rowe关系,可以有效提升本构模型的模拟效果,更准确地反映岩石材料的力学行为.
5. 结 论
1) 由于黏聚力影响,使水泥加固砂土和岩石等含黏聚力材料的应力-剪胀响应数据偏离经典的应力-剪胀关系模型预测值.
2) 本文所提出的改进Rowe应力-剪胀关系模型能有效反映常规三轴试验下岩石应力-剪胀响应的总体趋势,并能有效预测“回旋勾”现象.
3) 本文所提出的改进Rowe应力-剪胀关系在保证较少参数的同时,能够更好地反映不同围压下岩石的剪胀特性(剪胀角变化).
4) 将本文所提应力-剪胀关系应用于本构模型和岩石力学行为模拟中,可以更有效地反映岩石的变形特性,提供更准确的预测结果.
致谢: 感谢深圳市科创委可持续发展计划专项(KCXFZ202002011008532, KCXFZ20201221173207020)对本研究的资助;感谢Katarzyna Dołzyk-Szypcio博士和杨宏伟副教授关于数据处理的建议.
-
表 1 3种典型应力-剪胀关系
Table 1. Three classical stress-dilatancy rules
表 2 改进Rowe准则参数
Table 2. Parameters of modified Rowe rule
岩石 E b ξ ω 中粒砂岩 30 0.30 6.00 0.10 Eibenstock Ⅱ花岗岩 50 0.10 4.80 0.20 表 3 模拟所用参数
Table 3. Parameters for simulation
参数 取值 μ 0.23 κ 0.015 λ 0.15 M 1.10 pc0/MPa 70 v0 1.258 ξ 4 ω 0.15 E 0.05 b 20 -
[1] 梁明纯,苗胜军,蔡美峰,等. 考虑剪胀特性和峰后形态的岩石损伤本构模型[J]. 岩石力学与工程学报,2021,40(12): 2392-2401.LIANG Mingchun, MIAO Shengjun, CAI Meifeng, et al. A damage constitutive model of rock with consideration of dilatation and postpeak shape of the stress-strain curve[J]. Chinese Journal of Rock Mechanics and Engineering, 2021, 40(12): 2392-2401. [2] 陈静,高睿,刘洋泽鹏,等. 黏土脏污对道砟集料的应力-剪胀关系影响[J]. 西南交通大学学报,2022,57(6): 1201-1207.CHEN Jing, GAO Rui, LIU Yangzepeng, et al. Effect of clay contamination on stress-dilatancy relationships of ballast aggregate[J]. Journal of Southwest Jiaotong University, 2022, 57(6): 1201-1207. [3] 高彦芳,任战利,姜海龙,等. 考虑剪胀性和应变软化的油砂非线性弹性模型[J]. 地下空间与工程学报,2023,19(1): 43-50.GAO Yanfang, REN Zhanli, JIANG Hailong, et al. A nonlinear elastic model for oil sands considering shear dilation and strain softening[J]. Chinese Journal of Underground Space and Engineering, 2023, 19(1): 43-50. [4] 寇昊,何川,陈子全,等. 考虑残余强度的层状岩体损伤演化规律[J]. 西南交通大学学报,2023,58(5): 1064-1072.KOU Hao, HE Chuan, CHEN Ziquan, et al. Damage evolution law of layered rock mass considering residual strength[J]. Journal of Southwest Jiaotong University, 2023, 58(5): 1064-1072. [5] 李修磊,陈洪凯,张金浩. 考虑初始空隙压密的岩石变形全过程本构模型[J]. 西南交通大学学报,2022,57(2): 314-321.LI Xiulei, CHEN Hongkai, ZHANG Jinhao. Statistical damage model for whole deformation and failure process of rock considering initial void closure[J]. Journal of Southwest Jiaotong University, 2022, 57(2): 314-321. [6] ROWE P W. The stress-dilatancy relation for static equilibrium of an assembly of particles in contact[J]. Proceedings of the Royal Society of London: Series A Mathematical and Physical Sciences, 1962, 269(1339): 500-527. [7] ZHANG J, SALGADO R. Stress-dilatancy relation for Mohr–Coulomb soils following a non-associated flow rule[J]. Géotechnique, 2010, 60(3): 223-226. [8] ROSCOE K H, SCHOFIELD A N. Mechanical behaviour of an idealized'wet'clay[C]//Proc 3rd Eur Conf Soil Mech. Wiesbaden: [s.n.], 1963: 47-54. [9] TAYLOR D W. Fundamentals of soil mechanics[M]. New York: Wiley, 1948. [10] MUIR WOOD D. Soil behaviour and critical state soil mechanics[M]. Cambridge: Cambridge University Press, 1990. [11] TSEGAYE A B, BENZ T. Plastic flow and state-dilatancy for geomaterials[J]. Acta Geotechnica, 2014, 9(2): 329-342. doi: 10.1007/s11440-013-0290-z [12] TSEGAYE A B. A new stress-dilatancy framework for the modelling of rocks and rock masses[J]. IOP Conference Series: Earth and Environmental Science, 2021, 727(1): 012014.1-012014.10. [13] JEFFERIES M. Plastic work and isotropic softening in unloading[J]. Géotechnique, 1997, 47(5): 1037-1042. [14] NOVA R. A constitutive model under monotonic and cyclicloading[C]//Soil mechanics—transient and cyclic loads. New York: John Wiley & Sons Ltd., 1982: 343-373. [15] XIAO Y, LIU H L, ZHU J G, et al. Dilatancy equation of rockfill material under the true triaxial stress condition[J]. Science China Technological Sciences, 2011, 54(1): 175-184. doi: 10.1007/s11431-010-4201-3 [16] 石北啸,刘赛朝,吴鑫磊,等. 考虑颗粒破碎的堆石料剪胀特性研究[J]. 岩土工程学报,2021,43(7): 1360-1366.SHI Beixiao, LIU Saichao, WU Xinlei, et al. Dilatancy behaviors of rockfill materials considering particle breakage[J]. Chinese Journal of Geotechnical Engineering, 2021, 43(7): 1360-1366. [17] 郭万里,朱俊高,彭文明. 粗粒土的剪胀方程及广义塑性本构模型研究[J]. 岩土工程学报,2018,40(6): 1103-1110.GUO Wanli, ZHU Jungao, PENG Wenming. Dilatancy equation and generalized plastic constitutive model for coarse-grained soils[J]. Chinese Journal of Geotechnical Engineering, 2018, 40(6): 1103-1110. [18] GUO W L, ZHU J G. Particle breakage energy and stress dilatancy in drained shear of rockfills[J]. Géotechnique Letters, 2017, 7(4): 304-308. [19] JIN W F, TAO Y, CHEN R Z. Capturing the turning hook of stress-dilatancy curve of crushable calcareous sand[J]. Journal of Marine Science and Engineering, 2022, 10(9): 1269.1-1269.19. [20] ALEJANO L R, ALONSO E. Considerations of the dilatancy angle in rocks and rock masses[J]. International Journal of Rock Mechanics and Mining Sciences, 2005, 42(4): 481-507. doi: 10.1016/j.ijrmms.2005.01.003 [21] WALTON G, ARZÚA J, ALEJANO L R, et al. A laboratory-testing-based study on the strength, deformability, and dilatancy of carbonate rocks at low confinement[J]. Rock Mechanics and Rock Engineering, 2015, 48(3): 941-958. doi: 10.1007/s00603-014-0631-8 [22] ZHAO R, LI C G. A new dilation angle model for rocks[J]. Rock Mechanics and Rock Engineering, 2022, 55(9): 5345-5354. doi: 10.1007/s00603-022-02835-6 [23] MAS D, CHEMENDA A I. Dilatancy factor constrained from the experimental data for rocks and rock-type material[J]. International Journal of Rock Mechanics and Mining Sciences, 2014, 67: 136-144. doi: 10.1016/j.ijrmms.2013.12.014 [24] RAHJOO M, EBERHARDT E. Development of a 3-D confinement-dependent dilation model for brittle rocks; part 1, derivation of a Cartesian plastic strain increments ratios approach for non-potential flow rules[J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 145: 104668.1-104668.27. [25] RAHJOO M, EBERHARDT E. Development of a 3-D confinement-dependent dilation model for brittle rocks; part 2, formulation and parameterization based on the Cartesian plastic strain increments ratios approach[J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 148: 104773.1-104773.30. [26] ZHAO X G, CAI M. A mobilized dilation angle model for rocks[J]. International Journal of Rock Mechanics and Mining Sciences, 2010, 47(3): 368-384. doi: 10.1016/j.ijrmms.2009.12.007 [27] CHANG C S, DENG Y B. Energy equation and stress-dilatancy relationship for sand[J]. Acta Geotechnica, 2022, 17(7): 2675-2696. doi: 10.1007/s11440-021-01389-1 [28] LIU J M, ZOU D G, KONG X J, et al. Stress-dilatancy of Zipingpu gravel in triaxial compression tests[J]. Science China Technological Sciences, 2016, 59(2): 214-224. doi: 10.1007/s11431-015-5919-8 [29] SZYPCIO Z, DOŁŻYK-SZYPCIO K. The stress-dilatancy behaviour of artificially bonded soils[J]. Materials, 2022, 15(20): 7068.1-7068.13. [30] WU S F, LI B, CHU J. Stress-dilatancy behavior of MICP-treated sand[J]. International Journal of Geomechanics, 2021, 21(3): 04020264.1-04020264.12. [31] TAN X, KONIETZKY H, FRÜHWIRT T. Numerical simulation of triaxial compression test for brittle rock sample using a modified constitutive law considering degradation and dilation behavior[J]. Journal of Central South University, 2015, 22(8): 3097-3107. doi: 10.1007/s11771-015-2846-6 [32] HU D W, ZHANG F, SHAO J F. Experimental study of poromechanical behavior of saturated claystone under triaxial compression[J]. Acta Geotechnica, 2014, 9(2): 207-214. doi: 10.1007/s11440-013-0259-y -





 下载:
下载:









 下载:
下载: