Shape Analysis of Main Cable of Single Tower Suspension Bridge with Unilateral Spatial Cable Plane and Curved Beam
-
摘要:
目前空间索面悬索桥主缆成桥线形分析方法需要进行复杂的主缆微分方程求解,具有约束方程形式复杂、迭代收敛性受初值影响大等不足. 为解决主缆线形求解过程收敛性问题,本文借鉴等代梁法思路,推导得到外荷载与主缆线形的几何关联方程,并进一步构造出求解空间主缆线形的两阶段分析方法:在粗算阶段,通过解耦处理将初值要求降到最低,得到“具有足够精度且确保收敛”的结果,作为精算阶段初值;在精算阶段,采用迭代计算得到空间主缆线形精确解. 通过人行悬索桥算例验证两阶段分析方法的可行性和有效性,并使用有限元软件验证计算成果精度. 研究成果表明:本文所提出的方法对初值要求低,无需专门构造初值,迭代循环剔除了变形相容条件及无应力长度计算,求解效率更高,并且能快速收敛得到主缆线形精确解,适用于单边空间索面曲梁独塔悬索桥主缆成桥线形分析.
Abstract:The current analysis method for the main cable shape of a suspension bridge with a spatial cable plane involves solving complex differential equations for the main cable. However, this approach has certain drawbacks, such as the complicated form of constraint equations and the significant influence of initial values on iterative convergence. To address these issues and improve convergence in determining the main cable shape, the equivalent beam method was used, and geometric correlation equations between external loads and the main cable shape were derived. Subsequently, a two-stage analysis method was developed to solve the spatial main cable shape: During the rough calculation stage, decoupling processing minimized initial value requirements while obtaining accurate results with sufficient convergence. These results were then used as initial values in the precise calculation stage to iteratively calculate an exact solution for the spatial main cable shape. The feasibility and effectiveness of this two-stage analysis method were demonstrated through an example involving a pedestrian suspension bridge, and finite element software was used to verify the accuracy of calculation results. The research results demonstrate that the proposed method exhibits lower requirements for initial values and does not require the setting of initial values. The iterative process eliminates deformation compatibility conditions and stress-free length calculation, resulting in enhanced solving efficiency and rapid convergence towards obtaining an accurate solution for the main cable shape. This approach is suitable for analyzing the main cable shape of a single tower suspension bridge with a unilateral spatial cable plane.
-
单边空间索面曲梁悬索桥具有造型简约优雅、通透感强等优点,是景区或城市重要节点人行桥选型有竞争力的方案,国内自2013年上海迪斯尼湖畔公园景观人行桥[1]建成后,陆续修建了多座相似桥梁[2-3].
在单边空间索面悬索桥成桥状态主缆线形分析过程中,主缆的线形、吊杆内力及方向均为未知量,且存在耦合,这使得主缆线形求解较为复杂.
传统的主缆计算理论(如抛物线理论、分段悬链线理论等)都是围绕主缆的微分方程展开研究,通过积分或者数值方法得到主缆线形[4]. 此过程一般假定水平力为固定值,并不适用于单边空间索面悬索桥主缆线形分析,因为斜吊杆等会引起主缆水平力改变.
关于空间索面的悬索桥主缆成桥线形分析的研究相对较少,主要是基于分段悬链线的空间分段悬链线法和数值解析法. 罗喜恒等[5]将分段悬链线法进行拓展,用以求解空间索面的悬索桥主缆. 彭苗等[6-9]以空间分段悬链线理论为基础,对收敛困难问题进行优化. 李传习等[10-11]提出空间缆索悬索桥数值解析法,以索段状态方程、索段平衡方程式和分点平衡条件构成空间主缆精确找形基本方程,结合搜索合适起始端初始内力,通过迭代完成对主缆的找形计算. 张文明等[12-13]提出基于分段悬链线理论和非线性GRG (generalized reduced gradient)法的主缆线形解析计算方法. 空间分段悬链线法和基于分段悬链线的数值解析法参数少,收敛速度快,是目前计算精度最高、应用最普遍的方法,但这类方法原理上需要引入包含索段无应力长度及变形相容条件的索段状态方程,由于缆索结构的非线性,使得初值的选择对迭代收敛性及收敛速度的影响较大.
马召宇等[14-16]基于ANSYS有限元模型,构造坐标迭代模型,求解主缆线形. 坐标迭代法和空间分段悬链线法存在共性的问题:在迭代过程中应用变形相容条件,缆索结构强烈的非线性对初值要求较高.
孙远等[17]提出“合理轴线”思路,基于桁架单元和结构力学的基本原理,建立单元坐标与杆端力的关系式;刘超等[18]提出基于整体力学分析的主缆坐标体系法,分别对塔顶、地锚点及主跨跨中取矩,建立整体力学平衡方程,求解地锚支点反力,再通过迭代求解出主缆线形坐标. 这2种思路都将无应力长度及变形相容条件后置,大大降低对初值的要求.
由上述研究可知,无应力长度及变形相容条件不是求解主缆线形的必要条件,而且如果将其后置,解除缆索长度变形与空间变位的耦合关系,将大大降低对迭代初值的要求,提高算法普适性.
本文借鉴等代梁法思路[19],基于荷载与主缆几何线形的关联方程,用数值迭代求解主缆线形,并将计算流程拆分成粗算和精算2个阶段. 其中,粗算阶段通过合理简化,降低初值标准,既能提高算法普适性,又能得到“足够精度确保收敛”的精算阶段初值;精算阶段完成空间主缆线形的精确求解.
1. 空间主缆几何线形关联迭代法
1.1 基本假定与约定
分析主缆受力时采用如下假定:
1) 主缆为理想柔性索,不考虑其抗弯刚度及抗扭刚度;
2) 主缆受到的吊杆集中力只作用在节点处.
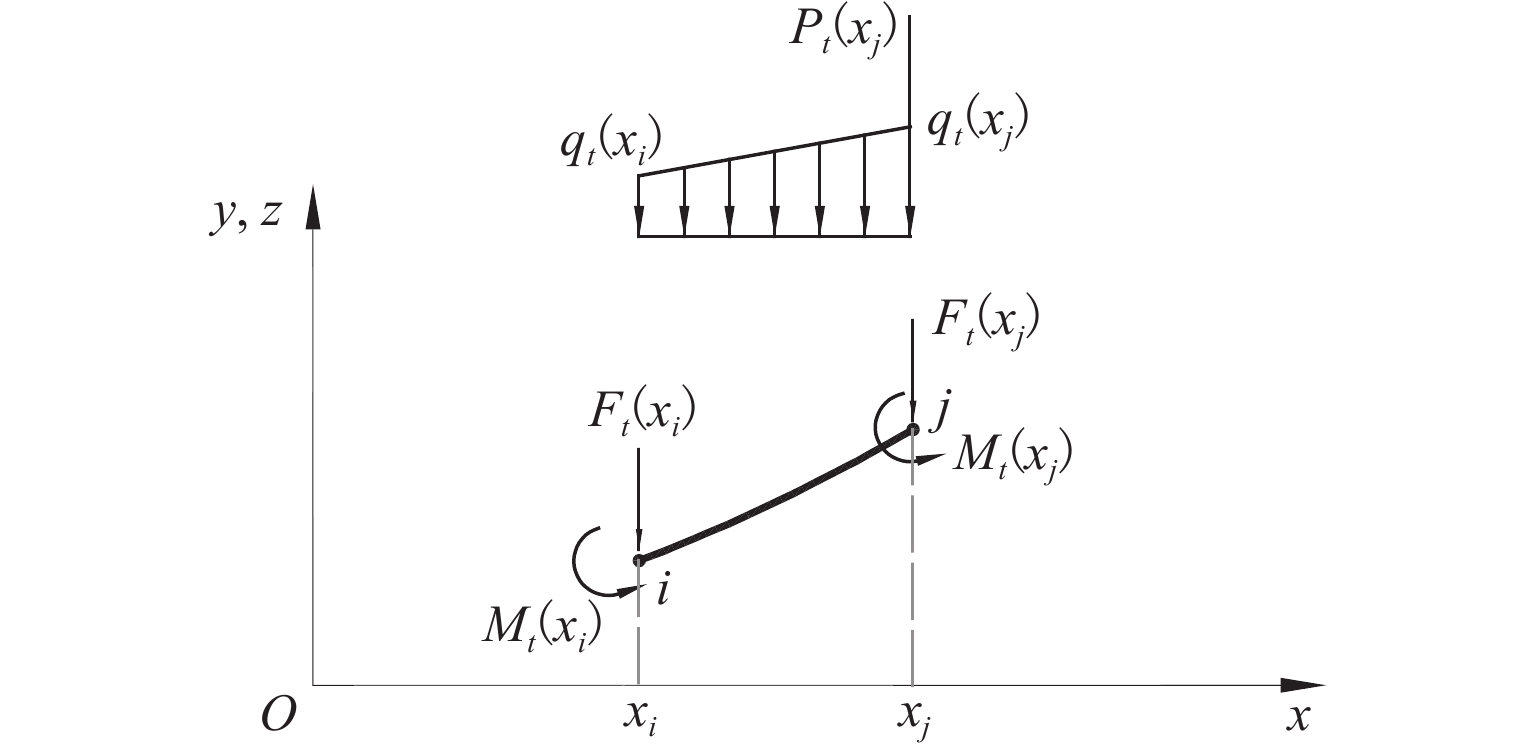
坐标系、力和力矩方向约定如图1所示,以地锚点为原点,与加劲梁的吊点连成的平面曲线的切线为x轴,竖直向上为z轴正向,按右手法则确定坐标系. 力和力矩的方向均以坐标轴同向为正. 点A为主缆在塔顶锚固点,点C为主缆跨中垂点,点B坐标(x,y,z),为主缆上任意点. 主缆跨径为L,两端点z轴方向高差为hz,y轴方向坐标差为hy,跨中垂度为fz. 成桥状态悬索桥主缆承受荷载有2种:沿主缆索长分布的均布荷载函数qt(x) (如主缆自重、主缆检修道等)及主缆受到的吊杆集中荷载函数Pt (x) (如通过吊杆传递的加劲梁恒载、索夹、吊杆及锚具自重等). 其中:下标$ t\in \left\{x,y,{\textit{z}}\right\} $表示荷载方向, Pt(xi)、Pt(xj)分别为作用在x轴xi、xj处的主缆集中力,Ftl为地锚点O支点反力.
1.2 外荷载节点力和力矩
定义主缆上任意节点左侧外荷载在该点产生的节点力为外荷载节点力Ft(x),产生的节点力矩为外荷载节点力矩Mt(x).
如图2所示,取任意空间主缆单元,计算单元右端的外荷载节点力为
Ft(xj)=Ft(xi)+qt(xi)+qt(xj)2Ki(xj−xi)+Pt(xj), (1) 式中:Ki为计算单元的荷载分布长度系数,分布荷载沿主缆索长分布时,近似计算如式(2)所示,其中,yi、yj和zi、zj分别为节点i、j的y轴和z轴坐标.
Ki=√1+(yj−yixj−xi)2+(zj−zixj−xi)2. (2) 假设作用在节点i的虚拟力Vt(xi) (式(3))在节点j产生的力矩与节点j处的外荷载力矩相等,将此虚拟力定义为外荷载力矩等代力.
Vt(xi)=Ft(xi)+2qt(xi)+qt(xj)6Ki(xj−xi). (3) 则外荷载节点力矩迭代式为
{Mx(xj)=Mx(xi)+Vy(xi)(zj−{\textit{z}}i)−Vz(xi)(yj−yi),My(xj)=My(xi)+Vz(xi)(xj−xi)−Vx(xi)(zj−zi),Mz(xj)=Mz(xi)+Vx(xi)(yj−yi)−Vy(xi)(xj−xi). (4) 由式(4)可见,任意方向力矩均可表示为三部分:前节点力矩、主方向力矩和耦合方向力矩. 当计算精度要求不高时,可通过略去较小的耦合方向力矩,实现对竖向z轴和水平y轴方向的解耦.
初始端边界值为
{Ft(0)=0,Mt(0)=0. (5) 1.3 主缆线形方程
根据理想柔索假定,主缆上任意点力矩均为0,因此,依次对主缆上任意点(x,y,z)的x、y、z轴方向取矩,可得
Fylz−Fzly+Mx(x)=0, (6) Fzlx−Fxlz+My(x)=0, (7) Fxly−Fylx+Mz(x)=0. (8) 消掉Fxl、Fyl、Fzl,得到荷载与主缆几何线形的关联方程为
Mx(x)x+My(x)y+Mz(x)z=0. (9) 定义主缆坐标的收敛完成率为
p=1−|Mx(x)x+My(x)y+Mz(x)z||Mx(x)x|+|My(x)y|+|Mz(x)z|. (10) 主缆坐标的收敛完成率是评价主缆坐标迭代计算完成度的指标.
如图1,主缆右端点坐标为(L,hy,hz),跨中垂点坐标为(L/2, y, fz),将右端点和跨中垂点的x、z轴坐标分别代入式(7),可得
{FzlL−Fxlhz+My(L)=0,FzlL2−Fxl(hz2−fz)+My(L2)=0. (11) 消元并整理后,求得地锚支点反力x轴向分力为
Fxl=My(L)−2My(L2)2fz. (12) 把主缆右端点坐标代入式(7)、(8),分别求得
Fzl=Fxlhz−My(L)L, (13) Fyl=Fxlhy+Mz(L)L. (14) 变换式(7)、(8),得到主缆线形方程为
{y=Fylx−Mz(x)Fxl,z=Fzlx+My(x)Fxl. (15) 将式(12)~(14)代入式(15),得到主缆线形方程的另一种表达式为
{y=2fzMy(L)−2My(L2)(Mz(L)Lx−Mz(x))+hyLx,z=−2fzMy(L)−2My(L2)(My(L)Lx−My(x))+hzLx. (16) 传统抛物线理论的主缆线形方程为式(16)在沿跨径方向均布荷载作用下的特例,主缆上任意点的外荷载节点力矩为
My(x)=12qx2. (17) 将式(17)代入式(16)的z轴坐标表达式,即可得到常见的主缆线形抛物线方程为
z=−4fzL2x(L−x)+hzLx. (18) 式(15)、(16)分别适用于编程和Excel表格计算.
1.4 主缆无应力长度
1.4.1 传统方法
传统分段悬链线法及数值解析法计算主缆无应力长度方法较为复杂,引用李传习等[20]成果如式(19)所示.
l=−HS0EA−Hq[ln(V+√H2+V2)−ln(V−qS0+√H2+(V−qS0)2)], (19) 式中:l为索段左右两端点水平距离,H、V分别为索段左端张力的水平分力、竖直分力,S0为索段的无应力长度,q为单位长度索段的重力,E为索段截面弹性模量,A为索段截面面积.
假设式(19)中除S0外,其余均为已知量,可通过迭代求解主缆的无应力长度. 实际求解过程中,索段张力分力H、V往往是未知量,同样需要迭代求解,主缆无应力长度的求解相当复杂.
1.4.2 几何关联迭代法
几何关联迭代法不需要使用变形相容条件求解线形,因此,可以将单元的无应力长度求解过程后置于主缆线形求解之后. 这一方面可以降低对初值要求,另一方面可以在求解无应力长度时通过临时加密计算节点数,提高计算精度至满足要求.
主缆节点i (xi, yi, zi)的节点张力分力为
Tti=Ftl+Ft(xi). (20) 主缆节点i的张力为
Ti=√T2xi+T2yi+T2zi. (21) 单元伸长量为
ΔLi=(Ti−1+Ti)Li2EA. (22) 单元无应力长度为
Lui=Li−ΔLi. (23) 主缆无应力长度S0计算式为
S0=S−∑iΔLi, (24) 式中:S为主缆的形状长度.
1.5 几何关联迭代法的应用
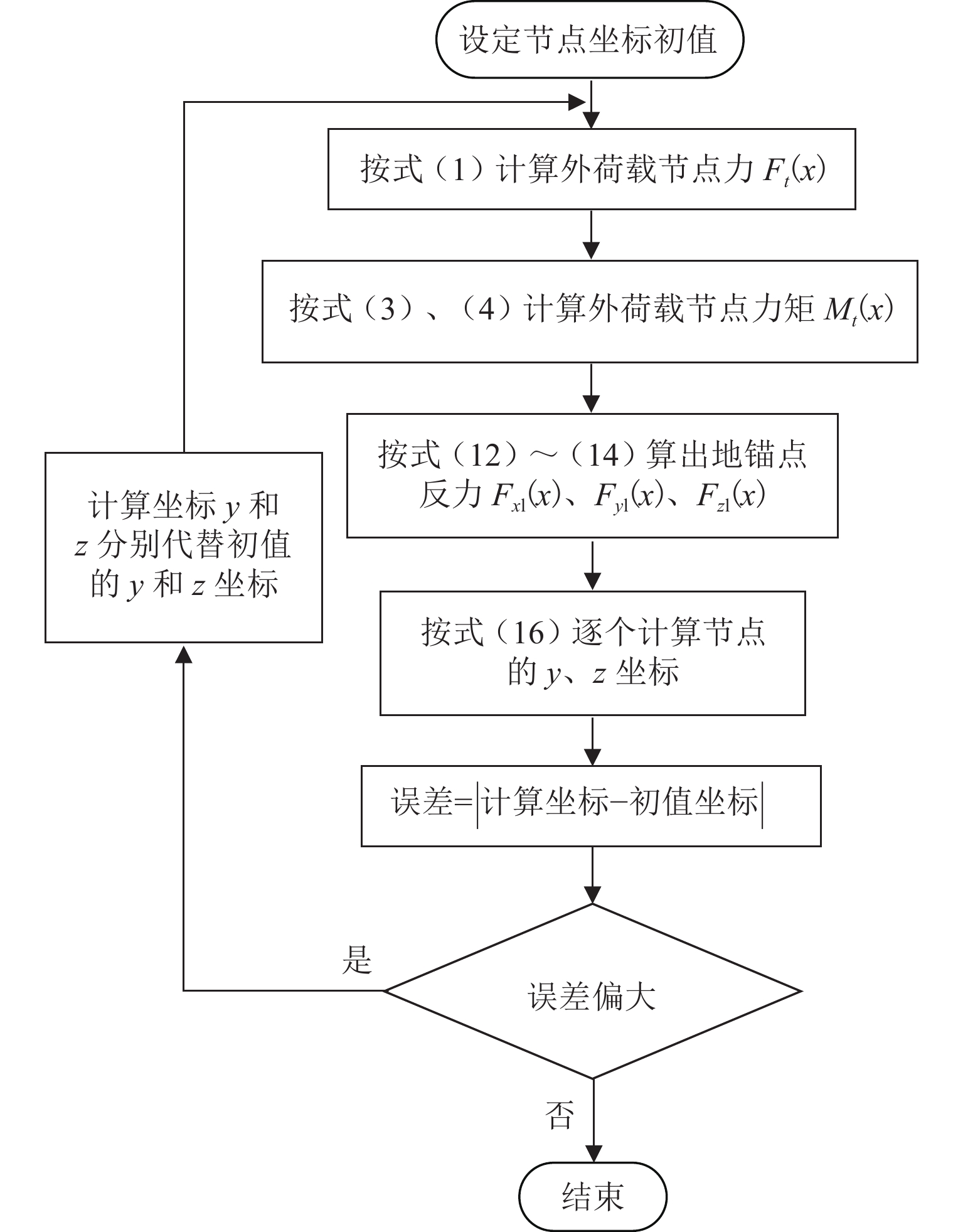
几何关联迭代法求解主缆线形需要如下参数:
1) 节点坐标的初值序列,其中,x坐标应满足递增,并完整覆盖主缆跨度L,粗算阶段y和z可随意取值,精算阶段使用粗算阶段坐标结果作为初值;
2) 主缆的外荷载qt (x)和Pt (x);
3) 主缆两端z轴方向坐标差hz、跨中垂度fz、主缆两端y坐标差hy (通常根据桥塔临近吊杆倾角、高度及用地情况确定).
具体计算流程如图3所示.
几何关联迭代法循环流程简单,迭代收敛速度快,适用于Excel或者编程计算.
2. 单边空间索面主缆线形计算
缺少边界条件的单边空间索面主缆的合理成桥状态并非唯一,需要引入合理的约束以得到符合设计意图的索形.
吊杆的竖向分力是平衡曲线主梁结构重力最有效的途径,故可将吊杆竖向力作为找形过程中的不变量,相应地将吊杆倾角作为自变量. 吊杆张力初始值按吊杆分担桥面面积对应的一期及二期恒载确定,并根据钢箱梁结构验算适当调整. 吊杆最小倾角取为0,即垂直吊杆,最大倾角由景观设计师综合考虑用地及造型确定.
主缆线形采用几何关联迭代法,计算过程存在嵌套迭代:吊杆倾斜角度与主缆坐标匹配度的外层迭代;固定吊杆倾斜角度后,计算主缆坐标的内层迭代. 计算过程的迭代复杂度是O(n2),对初值精度较为敏感,其中,n为迭代次数.
为降低初值需求、加速收敛,把计算流程分解成粗算阶段和精算阶段.
2.1 粗算阶段
粗算阶段通过合理简化,快速构造“足够精度确保收敛”的初值序列,主要措施如下:
1) 解耦:观察主缆线形方程(式(16))及外荷载力矩(式(4))可知,外荷载与线形的耦合关系是在计算外荷载力矩过程中引入的,可以通过对式(4)进行合理简化,消除耦合关系,将迭代复杂度降低成O(n). 略去吊杆在x轴方向分力,解除x轴方向力与y、z坐标耦合后,外荷载力矩My(x)只与z轴方向外荷载相关,Mz(x)只与y轴方向外荷载相关,主缆线形计算拆分成竖平面线形计算和水平面线形计算2个相互独立的模块. 解耦处理后y、z坐标计算过程不再需要迭代,实质上也不需要设置初值,只需依据节点划分设定x坐标,并确保x坐标满足递增并覆盖跨径L即可.
2) 集中荷载转分布荷载:粗算阶段需要通过调整吊杆倾角,达到收敛的目的,初始迭代时误差较大,如吊杆作为集中力调整,容易出现收敛计算反复横跳情况,将集中力转化为分布荷载后,大大降低荷载调幅难度,达到快速收敛的目的. 其中,吊杆竖直z向分力分散为梯形荷载,吊杆水平y向分力分散为曲边三角荷载,并均按吊杆间距分摊到主缆上.
3) 沿主缆索长方向的分布荷载简化为沿主缆跨径方向的分布荷载,消除分布荷载与主缆y、z坐标的耦合性.
2.1.1 竖平面线形计算
粗算阶段简化后,计算单元的荷载分布长度系数Ki按式(25)抛物线弧长公式计算,并取代式(2).
Ki=1+h2z2L2+8f2z3L2. (25) 吊杆力集中荷载转化为分布荷载后,主缆的竖平面分布荷载为
qz(x)=Kiqs+qd(x), (26) 式中:qs为主缆单位长度重量,按常规正桥单索面垂直吊杆模型测算主缆张力并选择主缆规格;qd(x)为吊杆集中荷载转化的分布荷载函数.
解耦后的竖平面线形计算无需迭代,坐标初值只需满足递增,并完整覆盖主缆跨度. 按图3流程即可求解竖平面线形.
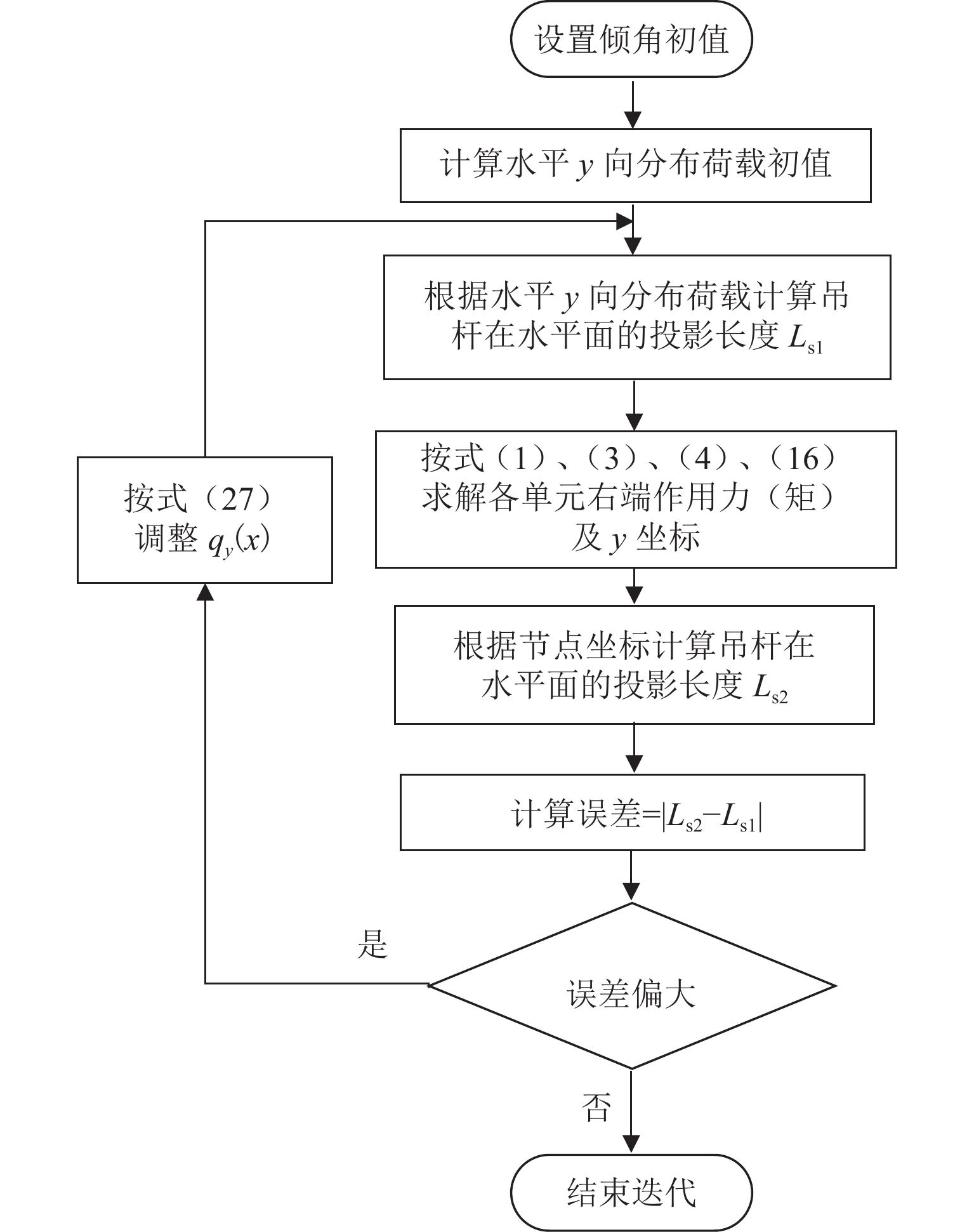
2.1.2 水平面线形计算
解耦后,固定荷载作用下的水平面线形计算同样无需迭代,原有的嵌套迭代只保留吊杆倾斜角度与主缆坐标匹配度的外层迭代. 迭代坐标初值x坐标与竖平面线形计算保持一致,y坐标随意,迭代流程如图4所示.
水平面线形计算围绕吊杆在水平面投影长度的计算构造迭代,投影长度计算有2种方法:
1) 根据y向分布荷载与竖向分布荷载比例求出吊杆倾角,再根据竖平面计算得到的吊杆高度求出吊杆在水平面投影长度Ls1;
2) 已知y向分布荷载,按式(1)~(13)求出吊杆在主缆上的吊点坐标,再结合桥面钢箱梁边缘的吊点坐标求出吊杆在水平面的投影长度Ls2.
迭代的收敛条件是上述2种方法计算的吊杆在水平面的投影长度差值符合精度要求.
迭代的变量是水平面y向分布荷载. 初始迭代轮次的水平面y向分布荷载根据吊杆初始倾角及吊杆竖向分布荷载计算确定,后续迭代轮次的y向分布荷载qy(x)均由前一迭代轮次计算采用值qyF(x)和荷载增量 ∆qy(x)组成,如式(27)所示.
qy(x)=qyF(x)+Δqy(x). (27) 荷载增量 ∆qy(x)采用前一迭代轮次计算的投影长度差值对应的荷载差值,并尽可能让当前迭代轮次的节点投影长度差低于前一迭代轮次最大误差2个数量级即可. 修改任意节点的荷载增量都会影响所有节点的y坐标,但是按上述原则保持单方向修改可以保证误差逐步收敛.
2.1.3 阶段成果
粗算阶段最终成果包括:由竖平面线形计算和水平面线形计算成果汇总成各节点坐标、竖向z轴和水平向y轴的分布荷载.
2.2 精算阶段
精算阶段应将粗算阶段成果中的吊杆分布荷载合并成集中荷载.
精算阶段需要进行嵌套迭代计算,其中,外层迭代是主缆坐标变化与吊杆倾斜度变化匹配迭代. 外层迭代流程如图5所示.
内层迭代是固定外荷载作用下的主缆线形计算,采用图3计算流程.
至此,通过粗算阶段和精算阶段的逐步迭代,最终完成主缆线形计算.
3. 算 例
如图6所示,厦门山海步道和美桥为单边索面独塔人行悬索桥,主跨216.7 m,采用V型桥塔,塔高53.56 m,桥塔菱形钢箱截面,最大尺寸2.0 m×1.6 m,根部通过球铰支座与基础连接,南北两段主缆以V塔为对称轴,主缆的低端锚固于地锚,高端锚固于V塔塔顶,V塔双柱之间设桥塔连接索,主缆采用全封闭高钒索,截面积A=129.69 cm2. 南北主缆各有17根吊索,吊索间距6 m. 主缆两端z轴方向坐标差hz=30 m、跨中垂度fz=9 m、主缆两端y坐标差hy=5 m.
人行桥面位于半径167.65 m的圆曲线范围内,采用钢箱梁,宽度4.4 m,梁高1.2 m,钢箱吊杆吊点偏心布置于钢箱外边缘.
坐标系以北缆地锚点为坐标原点,地锚点与首根吊杆的钢箱梁吊点在水平面上投影点连线为x轴方向,竖直向上为z轴方向,坐标轴方向满足右手定则.
3.1 粗算阶段
解耦后固定外荷载作用下的竖平面线形不用迭代即可直接求出坐标.
水平面线形计算需要根据图4流程迭代,寻找满足要求的水平荷载. 吊杆倾角初值的拟定综合考虑受力、景观及用地因素,本桥设计时,希望吊杆倾角呈现有节奏的变化,最靠近塔顶的吊杆倾斜角度控制在45° 左右,最矮的吊杆为垂直吊杆,中间的倾角按线性变化,根据吊杆倾角和吊杆竖向力可以求出水平分布荷载初值及吊杆的顶端点在水平面上的投影连线.
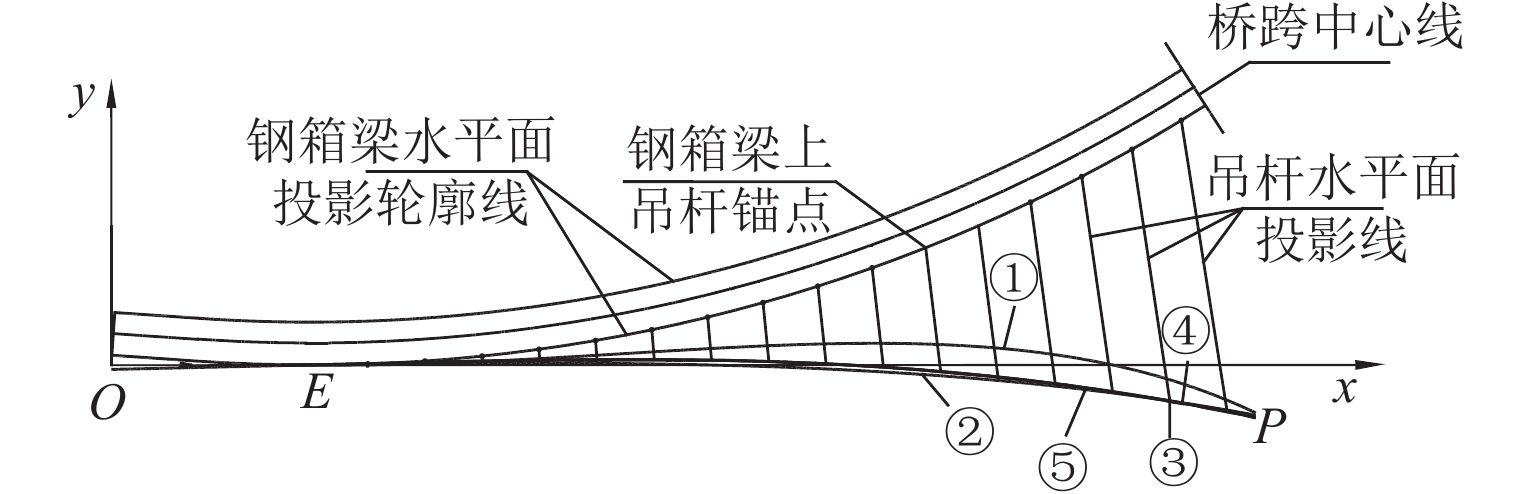
1/2桥平面投影如图7所示,点P是主缆在塔顶锚点在水平面的投影,点E是首根吊杆,坐标轴Ox与曲线钢箱梁在点E相切,①~⑤ 是投影线. ⑤ 线是最终主缆在水平面投影线,迭代过程如下:
第1轮迭代:根据倾角渐变原则逐一计算吊杆倾角,再通过竖平面线形计算得到主缆z坐标,即可计算出吊杆顶端点在水平面投影线 ① 线(即Ls1);根据吊杆的竖向力和倾角求出y方向分布荷载初值,按式(1)~(16)求出吊杆在主缆上的吊点坐标,结合桥面钢箱梁边缘的吊点坐标求出吊杆顶端点在水平面的投影线 ② 线(即Ls2),这2根线最大误差为3.38 m;
第2轮迭代:按式(27)对y向荷载进行调幅,由y向分布荷载与竖向分布荷载比值求出吊杆倾角,再结合主缆z坐标可求出荷载调幅后吊杆顶端点在水平面的投影线 ③ 线(即Ls1);再根据y向荷载计算出的主缆上吊点坐标,求出吊杆顶端点在水平面投影 ④ 线(即Ls2),2根线误差收敛到0.102 m. 后续轮次迭代模式均与第2轮次相同.
依次经过5轮迭代,最大误差依次为3380.2、101.8、15.6、1.6、0.2 mm,最终收敛到0.2 mm.
3.2 精算阶段
从粗算阶段转入精算阶段,吊杆荷载由分散荷载变成集中荷载,精算阶段首轮迭代保持荷载不变,直接进行内层迭代,修正坐标,首轮迭代坐标偏差组合值为6.2 cm.
精算阶段从第2轮迭代开始,均按图5流程先调荷载再调坐标.
精算阶段进行了5轮迭代,由于初值足够精确,所以每轮迭代都是内外层各计算一次,如表1 所示.
表 1 精算阶段迭代计算情况Table 1. Calculation iteration in precise calculation stage轮次 坐标偏差
组合值/mm吊杆 x 轴向
分力差值/kN吊杆 y 轴向
分力差值/kN1 62.0 0.0197 1.1095 2 1.0 0.0194 1.1095 3 0.1 0 0.0935 4 7.1×10−3 9.7000×10−8 0.0263 5 1.6×10−3 2.6000×10−8 0.0073 主缆各索段无应力长度Lui按式(23)计算,计算结果为S=124.2791 m,累计伸长量$\displaystyle\sum\nolimits_i^{} {} $ΔLi=0.1943 m,S0=124.0848 m.
3.3 与有限元软件成果对比
为验证计算方法的正确性,采用空间有限元软件Midas/Civil进行校验验算.
按精算阶段的成果输入主缆坐标,定义材料及截面,生成单元并设定索单元的初拉力,建立空间有限元模型,非线性分析收敛条件设定为10−6,进行非线性迭代分析计算,经过3轮迭代,Midas/Civil模型各节点位移收敛,最大组合位移误差小于0.1 mm.
对比几何关联迭代法结果和Midas/Civil计算结果可以看出,两者节点位置和张力差距很小,节点位置最大误差0.409 mm,节点张力最大误差0.14 kN,各节点位置和节点张力误差如图8所示.
4. 结 论
本文提出基于外荷载与主缆线形的几何关联方程迭代法求解空间主缆线形,研究空间主缆线形计算新的思路,将基于分段悬链线理论的计算方法中容易引起迭代收敛失败的变形相容条件从求解必要条件中剥离,简化了求解难度,提高算法普适性.
针对于空间主缆与吊索相互耦合,提出分阶段逐步逼近的思路,粗算阶段通过解耦降低初值要求,求得高质量的精算阶段初值,精算阶段通过迭代计算得到空间主缆线形精确解.
几何关联迭代法的特点既适用于普及率较高的Excel编公式迭代,也可通过编程作为通用有限元程序的插件,为有限元程序提供成果数据.
-
表 1 精算阶段迭代计算情况
Table 1. Calculation iteration in precise calculation stage
轮次 坐标偏差
组合值/mm吊杆 x 轴向
分力差值/kN吊杆 y 轴向
分力差值/kN1 62.0 0.0197 1.1095 2 1.0 0.0194 1.1095 3 0.1 0 0.0935 4 7.1×10−3 9.7000×10−8 0.0263 5 1.6×10−3 2.6000×10−8 0.0073 -
[1] 花炳灿. 悬浮在湖面上的飘带: 迪斯尼湖畔公园景观人行桥项目设计[J]. 建筑技艺,2014(5):84-86. [2] 李立斌,王立彬,李建慧. 单索面曲梁悬索人行桥主缆线形分析[J]. 铁道科学与工程学报,2017,14(10):2162-2169. doi: 10.3969/j.issn.1672-7029.2017.10.018LI Libin, WANG Libin, LI Jianhui. Cable shape analysis of curved suspension footbridge with single cable plane[J]. Journal of Railway Science and Engineering,2017,14(10):2162-2169. doi: 10.3969/j.issn.1672-7029.2017.10.018 [3] 屈红伟. 和美桥独斜塔单索面曲线悬索步道桥体系转换[J]. 福建建设科技,2022(6):108-112. doi: 10.3969/j.issn.1006-3943.2022.06.026QU Hongwei. Hemei bridge single leaning tower single cable plane curve suspension trail bridge system conversion[J]. Fujian Construction Science & Technology,2022(6):108-112. doi: 10.3969/j.issn.1006-3943.2022.06.026 [4] 唐茂林. 大跨度悬索桥空间几何非线性分析与软件开发[D]. 成都: 西南交通大学, 2003. [5] 罗喜恒,肖汝诚,项海帆. 空间缆索悬索桥的主缆线形分析[J]. 同济大学学报(自然科学版),2004,32(10):1349-1354. doi: 10.3321/j.issn:0253-374X.2004.10.017LUO Xiheng, XIAO Rucheng, XIANG Haifan. Cable shape analysis of suspension bridge with spatial cables[J]. Journal of Tongji University (Natural Science),2004,32(10):1349-1354. doi: 10.3321/j.issn:0253-374X.2004.10.017 [6] 彭苗. 自锚式悬索桥空间缆索系统的求解方法[J]. 土木工程与管理学报,2011,28(1):24-27. doi: 10.3969/j.issn.2095-0985.2011.01.006PENG Miao. Solution strategy for special spatial-cable self-anchored suspension bridge[J]. Journal of Civil Engineering and Management,2011,28(1):24-27. doi: 10.3969/j.issn.2095-0985.2011.01.006 [7] 齐东春,沈锐利. 悬索桥空间缆索主缆线形的计算方法[J]. 铁道建筑,2013(4):13-16 doi: 10.3969/j.issn.1003-1995.2013.11.05QI Dongchun, SHEN Ruili. Calculation method of main cable shape-finding of suspension bridge with spatial cables[J]. Railway Engineering,2013(4):13-16. doi: 10.3969/j.issn.1003-1995.2013.11.05 [8] 吴月星,周建庭,孙马,等. 空间异形索面悬索桥主缆成桥线形计算方法[J]. 桥梁建设,2020,50(5):37-43. doi: 10.3969/j.issn.1003-4722.2020.05.006WU Yuexing, ZHOU Jianting, SUN Ma, et al. Geometry calculation method for main cables of completed suspension bridge with irregular spatial cable planes[J]. Bridge Construction,2020,50(5):37-43. doi: 10.3969/j.issn.1003-4722.2020.05.006 [9] 邢德华,刘化涤. 基于MATLAB空间索面自锚式悬索桥主缆成桥线形精确计算[J]. 公路,2019,64(6):131-135. [10] 李传习. 现代悬索桥静力非线性理论与实践[M]. 北京: 人民交通出版社,2014: 49-67. [11] 李传习,柯红军,刘海波,等. 空间主缆自锚式悬索桥成桥状态的确定方法[J]. 工程力学,2010,27(5):137-146.LI Chuanxi, KE Hongjun, LIU Haibo, et al. Determination of finished bridge state of self-anchored suspension bridge with spatial cables[J]. Engineering Mechanics,2010,27(5):137-146. [12] 张文明,常佳琦,田根民,等. 不等主跨三塔空间缆索悬索桥主缆线形解析计算方法[J]. 桥梁建设,2022,52(1):109-115. doi: 10.3969/j.issn.1003-4722.2022.01.015ZHANG Wenming, CHANG Jiaqi, TIAN Genmin, et al. An analytical method for main cable shape of three-tower suspension bridge with asymmetrical two main spans and spatial cables[J]. Bridge Construction,2022,52(1):109-115. doi: 10.3969/j.issn.1003-4722.2022.01.015 [13] ZHANG W M, CHANG J Q, TIAN G M. FEM-based shape-finding and force-assessment of suspension bridges via completed loop adjustment[J]. Journal of Bridge Engineering,2022,27(1):4021098.1-4021098.15. [14] 马召宇,许福友,檀永刚. 悬索桥空间缆索实用找形方法[J]. 计算力学学报,2021,38(5):651-657. doi: 10.7511/jslx20200812002MA Zhaoyu, XU Fuyou, TAN Yonggang. A practical method for shape finding of spatial cable curves of suspension bridges[J]. Chinese Journal of Computational Mechanics,2021,38(5): 651-657. doi: 10.7511/jslx20200812002 [15] SONG C L, XIAO R C, SUN B. Improved method for shape finding of long-span suspension bridges[J]. International Journal of Steel Structures,2020,20(1):247-258. doi: 10.1007/s13296-019-00283-7 [16] 李保俊. 柔性人行悬索桥空间索面抗风缆索系统的找形计算方法[J]. 石家庄铁道大学学报(自然科学版),2020,33(1):25-30,81.LI Baojun. Calculation method for the shape-finding of spatial wind cable system on flexible pedestrian suspension bridge[J]. Journal of Shijiazhuang Tiedao University (Natural Science Edition),2020,33(1): 25-30,81. [17] 孙远,罗文孝,刘梅. 基于有限元列式的悬索桥主缆找形算法[J]. 土木工程与管理学报,2018,35(2):116-123. doi: 10.3969/j.issn.2095-0985.2018.02.018SUN Yuan, LUO Wenxiao, LIU Mei. Shape-finding algorithm of the main cable system of suspension bridges based on finite element formulation[J]. Journal of Civil Engineering and Management,2018,35(2):116-123. doi: 10.3969/j.issn.2095-0985.2018.02.018 [18] 刘超,高展. 基于整体力学分析的自锚式悬索桥主缆找形法[J]. 同济大学学报(自然科学版),2020,48(1):1-6. doi: 10.11908/j.issn.0253-374x.19131LIU Chao, GAO Zhan. A method for determining cable shape of a self-anchored suspension bridge based on an overall mechanical analysis[J]. Journal of Tongji University (Natural Science),2020,48(1): 1-6. doi: 10.11908/j.issn.0253-374x.19131 [19] 项海帆. 高等桥梁结构理论[M]. 2版. 北京: 人民交通出版社,2013: 419-421. [20] 李传习,刘光栋,柯红军. 悬索桥主缆系统数值解析法计算的一种收敛算法[J]. 工程力学,2008,25(7):66-73.LI Chuanxi, LIU Guangdong, KE Hongjun. A convergent algorithm for numerical-analytic method of calculating main cable system of suspension bridge[J]. Engineering Mechanics,2008,25(7): 66-73. 期刊类型引用(0)
其他类型引用(1)
-






 下载:
下载:








 下载:
下载: