Time-Dependent Reliability Analysis of LS-FA-211001 Suction Anchor Under Cyclic Load
-
摘要:
为有效评估在使用过程中LS-FA-211001吸力锚的可靠性水平,考虑外部载荷的累积效应,建立循环载荷作用下的时变可靠性模型;结合LS-FA-211001吸力锚的不确定性量化数据,对其展开时变可靠性分析;利用蒙特卡罗模拟(MCS)方法对吸力锚可靠度进行验证. 结果表明:在可靠度要求95%以上的条件下,LS-FA-211001吸力锚的寿命即使在恶劣勘探点也能达到100次;同时,在循环载荷不同作用次数下,本文建立的吸力锚时变可靠性模型与MCS方法评估出的可靠度结果相比,误差不超过2.15%,验证了本文方法的有效性.
Abstract:To effectively evaluate the reliability level of the LS-FA-211001 suction anchor during application, the cumulative effect of external loads was considered, and a time-dependent reliability model was established under cyclic loads. The time-dependent reliability analysis of the suction anchor was carried out based on the quantified uncertainty data. The Monte Carlo simulation (MCS) method was adopted to verify the reliability of the suction anchor. The results show that the life of LS-FA-211001 suction anchor can reach 100 times even in harsh exploratory points under the reliability of being over 95%. At the same time, under different cycles of load, the error of the time-dependent reliability model of the suction anchor established in this paper is less than 2.15% compared with the reliability results evaluated by the MCS method, which verifies the effectiveness of the proposed method.
-
吸力锚系统主要由吸力锚、泵机组、应急解脱系统和控制系统组成,是集机械、电气、液压、控制于一体的复合系统[1-3]. 作为一种新型的海洋工程基础,吸力锚系统的可靠性直接关系到发射平台的可靠性、稳定性以及发射任务能否顺利进行等[4]. 然而,吸力锚系统在实际工程中往往受到循环载荷作用,针对循环载荷产生的累积效应,传统的基于应力-强度干涉模型的静态可靠性方法无法准确地给予描述. 因此,考虑吸力锚系统在循环载荷作用下的累积效应,开展时变可靠性研究十分必要.
目前,国内外学者对时变可靠性分析理论方法进行了很多研究并取得了丰硕的研究成果;Rice[5]在分析随机噪声时创造性地提出了首次跨越概率计算方法,为时变可靠性分析奠定了基础;Crandall等[6]利用数值分析方法求解了跨越率;Andrieu-Renaud等[7]针对跨越率的求解提出了PHI2方法,对时变可靠性的求解提供了一种高效的计算方法;Singh等[8]针对非单调功能函数的累积失效概率计算提出了一种改进的PHI2算法;Hu等[9-10]提出了一种精度更高的联合跨越率的求解方法;Jiang等[11]研究了基于随机过程离散的时变可靠性分析方法;Li等[12]基于Gamma过程研究了数控机床刀具的时变可靠性问题;Cazuguel等[13]研究了在随机载荷作用下的平板时变可靠性模型;Kopustinskas等[14]基于随机过程的微分方程研究了动态可靠性建模方法;张德权等[15]引入区间理论处理模型中参数不确定性问题,进而提出了I-PHI2方法近似求解时变可靠性模型. 更多的时变可靠性分析理论方法参见文献[16-19].
时变可靠性目前已得到了广泛研究,但大都在理论层面,实际工程应用方面还相当欠缺. 基于此,本文以LS-FA-211001吸力锚为例,考虑循环载荷作用的累积效应,开展吸力锚的时变可靠性研究. 首先,基于广义应力-强度干涉理论,建立循环载荷作用下的时变可靠性模型;其次,结合LS-FA-211001吸力锚抗拔力和锚链拉力的不确定性量化数据,对其开展时变可靠性分析;最后,利用蒙特卡罗模拟(MCS)方法对本文结果进行对比验证.
1. 循环载荷作用下的时变可靠性模型
传统的结构可靠性模型通常采用广义应力-强度干涉模型,即在外部载荷作用下产生的应力超过强度时,结构失效,通常将应力和强度描述为随机变量,从而基于概率分布得到应力小于强度的概率,即为可靠度. 当用fs(s)、Fs(s)分别表示应力随机变量s的概率密度函数和累积分布函数,fσ(σ)、Fσ(σ)分别表示强度随机变量σ的概率密度函数和累积分布函数时,结构的可靠度表示为
R=P(s<σ)=∫+∞−∞fσ(σ)∫σ−∞fs(s)dsdσ=∫+∞−∞fσ(σ)Fs(σ)dσ. (1) 值得指出的是,式(1)是结构在单一失效模式下的可靠性模型,而且该模型描述的是结构在某一个具体时刻的可靠度,并没有表达出结构在外部载荷循环作用下的累积效应,是一个静态的可靠性模型.
当考虑外部循环载荷的累积效应时,结构的可靠性模型(即时变可靠性模型)可以表述为
R(w)=P(si<σi, ∀i=1,2,⋯,w), (2) 式中:w为外部循环载荷次数,si为外部循环载荷在第i次作用时的应力随机变量,σi为外部循环载荷在第i次作用时的强度随机变量.
此时,应力和强度表现为随作用次数变化的离散随机过程. 显然,式(2)考虑了结构在外部循环载荷作用下的累积效应,即结构在外部循环载荷作用w次的可靠性模型不再是简单地计算第w次应力小于强度的概率,而是要保证外部循环载荷在w次作用中任何一次作用应力都要小于强度.
当外部循环载荷来源于同一个随机变量,且在不考虑结构强度退化的情况下,有:
R(w)=P(si<σi, ∀i=1,2,⋯,w)=P(s1<σ,⋯,sw<σ)=P(smax (3) 式中:{s_{\max }} = \max \{{s_1},{s_2},\cdots,{s_w}\},为最大顺序统计量.
由于{s_1},{s_2},\cdots,{s_w}是来源于同一个随机变量循环载荷作用下的广义应力,因此,可以把{s_1},{s_2},\cdots,{s_w}看作是来源于同一母体的样本观测值. 由概率论[20]可知,{s_{\max }}概率分布函数{f_{{s_{\max }}}}({s_{\max }})和累积分布函数{F_{{s_{\max }}}}({s_{\max }})分别为
{f_{{s_{\max }}}}({s_{\max }}) = w{({F_s}(s))^{w - 1}}{f_s}(s), (4) {F_{{s_{\max }}}}({s_{\max }}) = {({F_s}(s))^w}. (5) 此时,结合传统的广义应力-强度干涉模型可得外部循环载荷作用下的结构在单一失效模式且强度不退化下的时变可靠性模型,如式(6).
\begin{split} & R(w) = P({s_{\max }} < \sigma ) = \int_{ - \infty }^{ + \infty } {{f_\sigma }(\sigma ){F_{{s_{\max }}}}(\sigma ){\mathrm{d}}\sigma }= \\ &\quad \int_{ - \infty }^{ + \infty } {{f_\sigma }(\sigma ){{({F_s}(\sigma ))}^w}{\mathrm{d}}\sigma } . \end{split} (6) 2. 吸力锚的时变可靠性分析
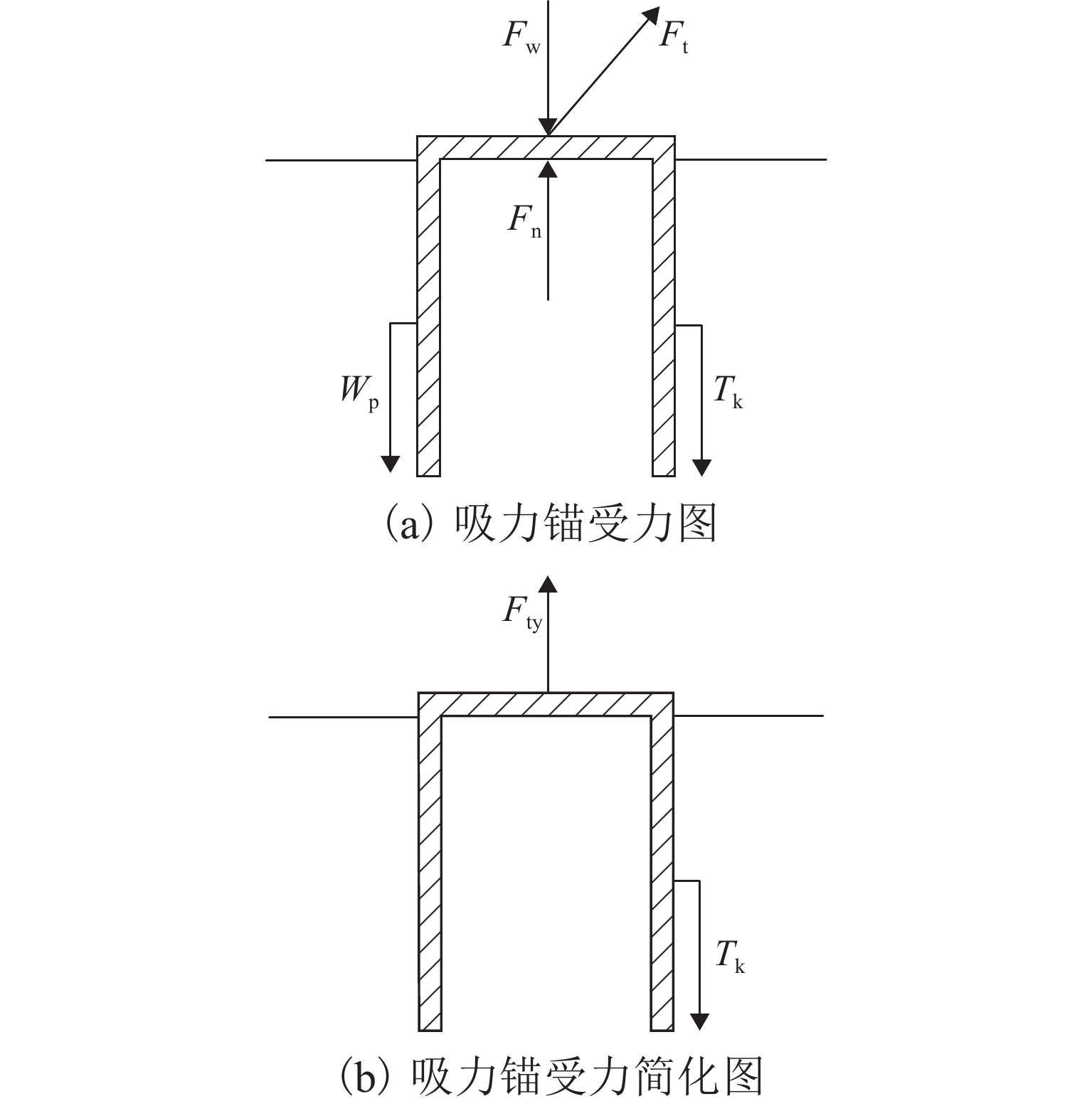
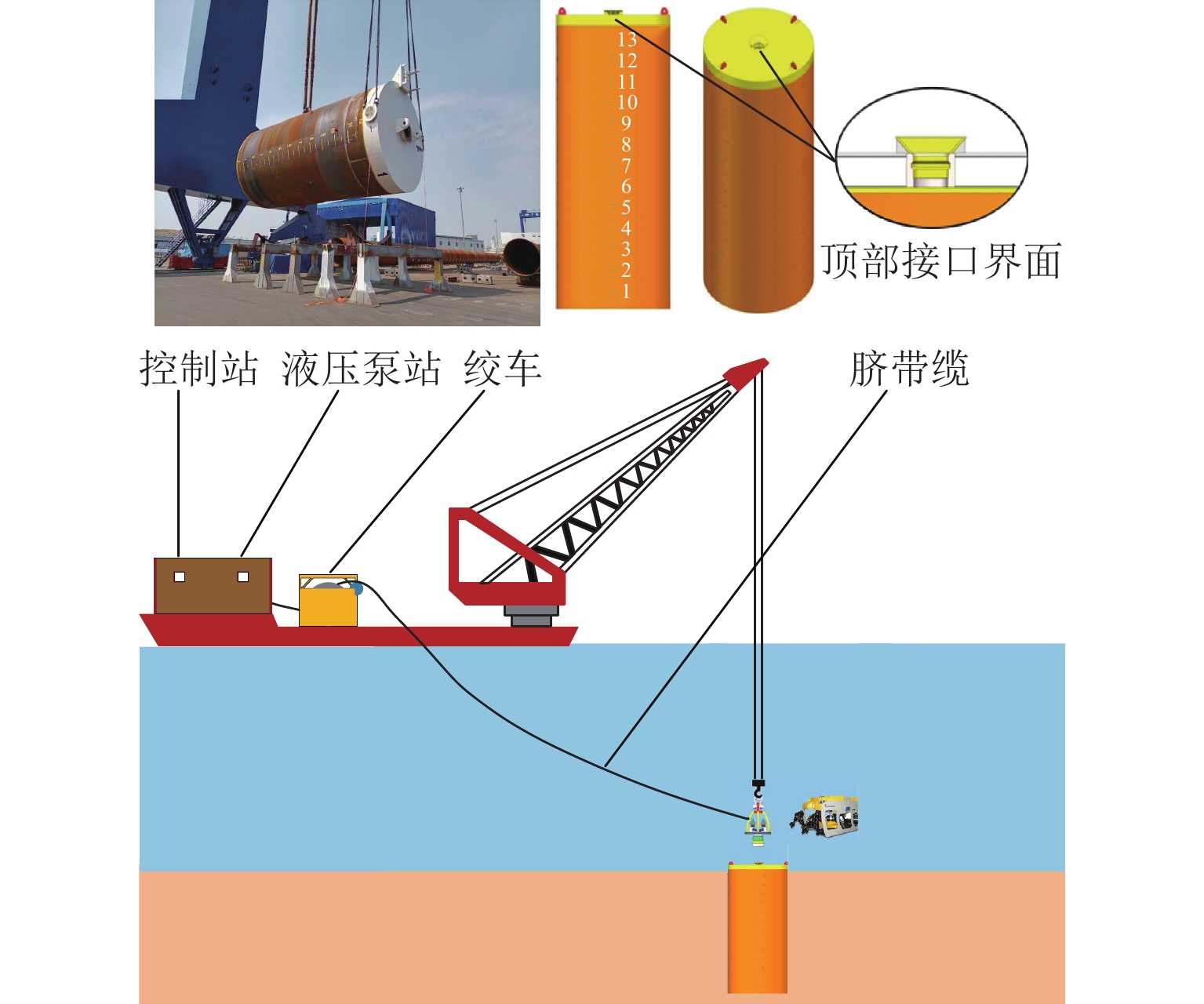
在实际工程中,LS-FA-211001吸力锚及其系统结构如图1所示,其承受着复杂的载荷,包括自身重力、土体地基的承载力、锚体侧壁与土体的摩擦力、锚链的拉力以及风、浪、流等的综合作用等. 由于条件的限制,前往锚泊现场进行水文环境监测和调研不具备可行性. 在此情形下对吸力锚展开精确的载荷分析存在困难. 因此,本文将吸力锚所受载荷进行简化,如图2所示.
在图2中,吸力锚主要受力包括:水对顶盖的压力{F_{\mathrm{w}}}、自身重力{W_{\mathrm{p}}}、土体的竖向承载力{F_{\mathrm{n}}}、锚链拉力{F_{\mathrm{t}}}以及筒壁侧摩阻力即抗拔力{T_{\mathrm{k}}}. 在安装的沉贯阶段,吸力锚尚未连接平台,此时吸力锚在自身重力、土体的竖向承载力和水的压力作用下逐渐达到静力平衡状态. 若只考虑竖直方向载荷,则吸力锚与平台建立连接后受力可简化为锚链拉力的竖直分量{F_{{\mathrm{ty}}}}和抗拔力{T_{\mathrm{k}}}.
2.1 抗拔力的不确定性量化
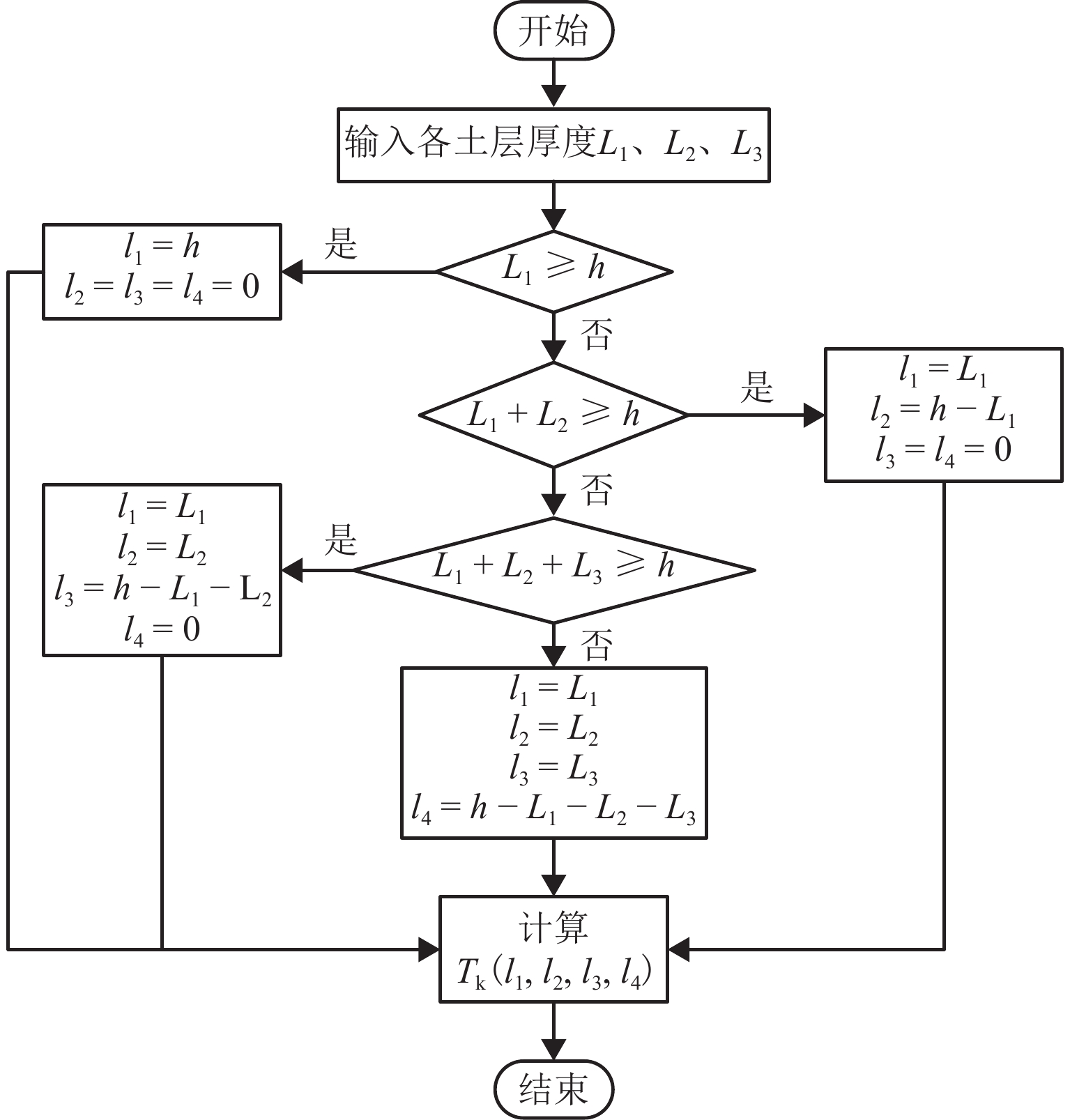
本文收集并计算了5个不同勘探点(点A、C、G、M、O)处的10组抗拔力数据,具体计算结果如表1所示. 此外,不同勘探点的平面图以及抗拔力计算流程分别如图3、4所示. 其中:{l_1}、{l_2}、{l_3}和{l_4}依次为某勘探点处吸力锚完全吸附时与淤泥质土、淤泥混砂、粉土-粉质黏土及粉细砂4个土层的接触厚度,{L_1}、{L_2}和{L_3}分别为淤泥质土、淤泥混砂及粉土-粉质黏土3个土层的实际厚度,h为给定值,本文取值13.5.
表 1 各勘探点附近的抗拔力值Table 1. Pulling resistance values near each exploratory pointkN 组号 点A 点C 点G 点M 点O 1 1330.9777 2007.6882 2247.3961 1826.8866 1835.3776 2 1339.7254 1998.8052 2261.1515 1820.1196 1840.0185 3 1336.7282 1982.7419 2274.1373 1814.4576 1843.6222 4 1330.8861 1964.7617 2286.1875 1809.4007 1846.4546 5 1323.8135 1947.6050 2297.1344 1804.6813 1848.9262 6 1319.7456 1954.0500 2191.5069 1800.0756 1851.5450 7 1319.9079 1970.4304 2187.9786 1795.7828 1854.8203 8 1322.8437 1987.0357 2182.8988 1792.0530 1859.1009 9 1328.1747 2003.6953 2176.1155 1791.3837 1864.3240 10 1338.2259 2020.2961 2167.4843 1791.0338 1869.6512 实际工程中吸力锚的抗拔力与其载荷环境息息相关,其计算过程涉及到土层厚度等多种地勘数据,相对复杂. 为了节约篇幅,本文直接给出了5个不同勘探点处的抗拔力计算结果,其详细计算流程及载荷环境的相关原始地勘数据可以参考文献[21]. 进一步利用K-S检验,可以判断该类型吸力锚的抗拔力服从正态分布,其均值和方差具体计算为
u = \frac{1}{n}\sum\limits_{j = 1}^n {{x_j}}, (7) {\sigma ^2} = \frac{1}{n}\sum\limits_{j = 1}^n {{{\left( {{x_j} - u} \right)}^2}}, (8) 式中:{x_j}为用于计算某勘探点处抗拔力的样本,n为样本总量.
针对计算的不同勘探点处的原始抗拔力数据,可以估算出其对应的不确定性参数,如表2所示.
表 2 各勘探点附近抗拔力分布参数Table 2. Parameters of pulling resistance distribution near each exploratory point勘探点 u \sigma A 1329.1029 7.4612 C 1983.7110 24.2016 G 2227.1991 48.0464 M 1804.5875 12.7894 O 1851.3841 10.7934 基于上述估算的不确定性参数可以获得不同勘探点处抗拔力的正态分布概率密度函数和累积分布函数,分别如式(9)、(10).
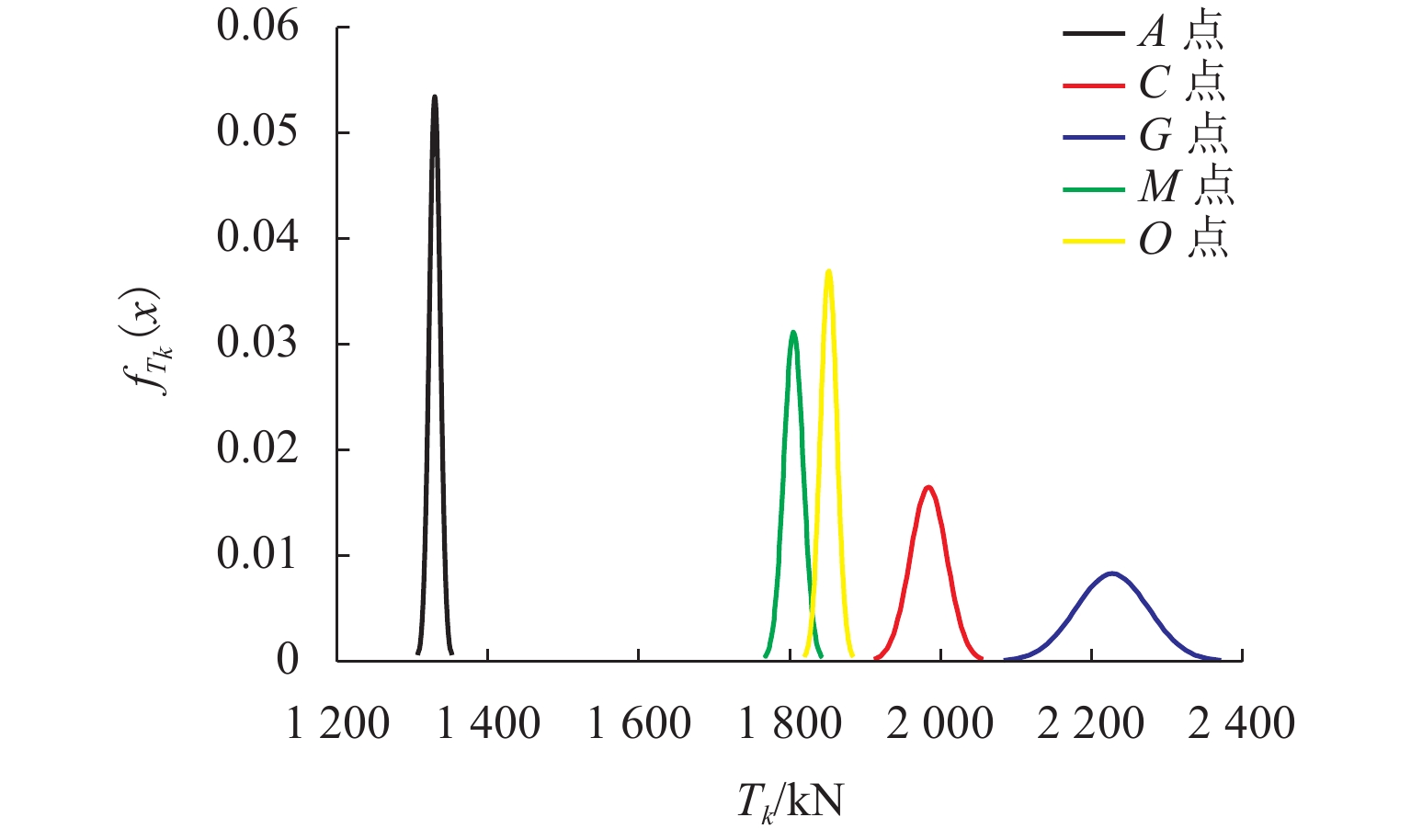
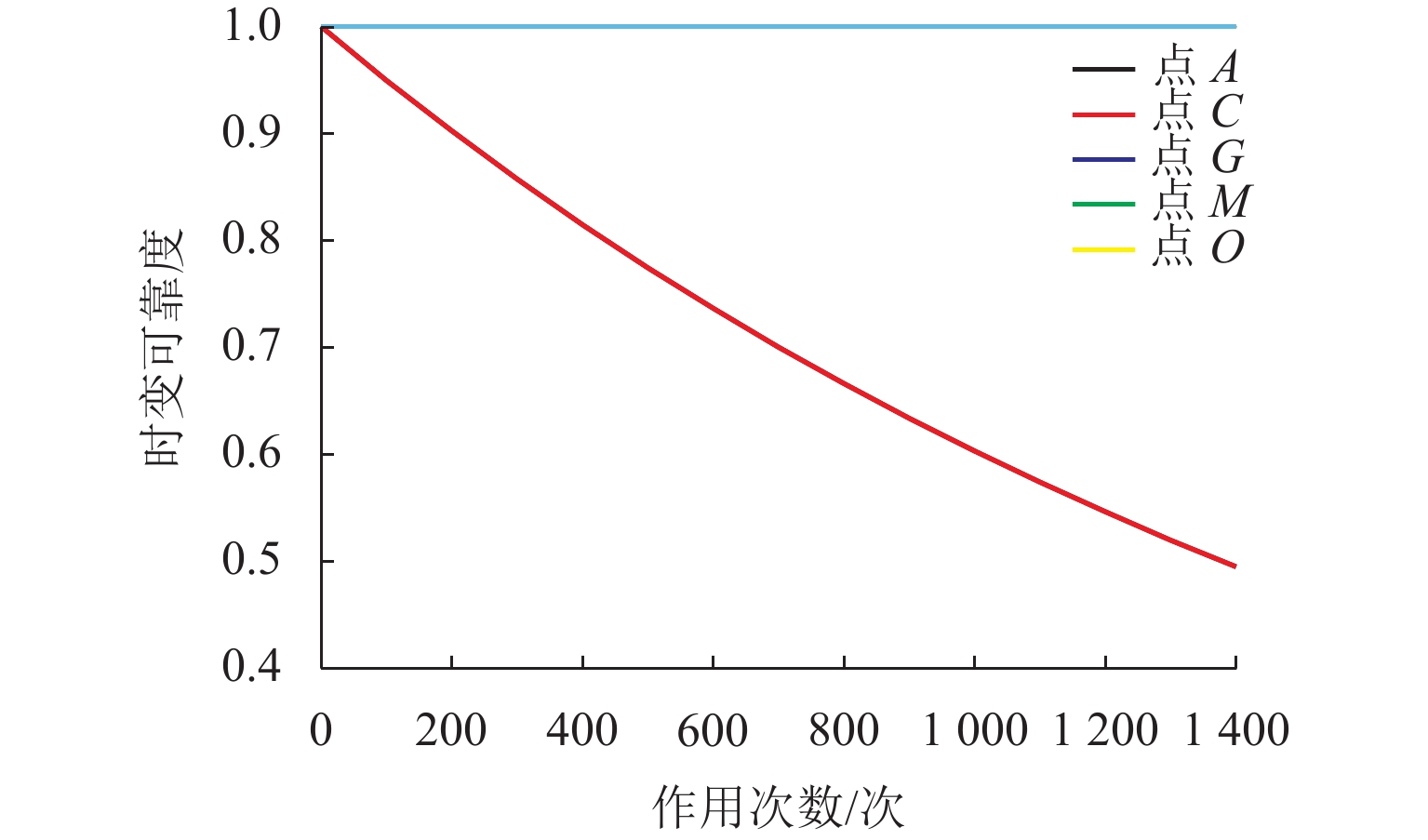
{f_{{T_{\mathrm{k}}}}}(x) = \frac{1}{{\sqrt {2{\text{π}} } \sigma }}{{\mathrm{e}}^{ - \frac{1}{2}{{\left( {\frac{{x - u}}{\sigma }} \right)}^2}}}, (9) {F_{{T_{\mathrm{k}}}}}(x) = \int_{ - \infty }^x {\frac{1}{{\sqrt {2{\text{π}}} \sigma }}{{\mathrm{e}}^{ - \frac{1}{2}{{\left( {\frac{{x - u}}{\sigma }} \right)}^2}}}{\mathrm{d}}x}. (10) 不同探勘点处量化的抗拔力正态分布概率密度函数如图5所示. 从图中可知,点A处的抗拔力最小,说明该勘探点处的吸力锚是最容易失效的.
2.2 吸力锚时变可靠性计算结果
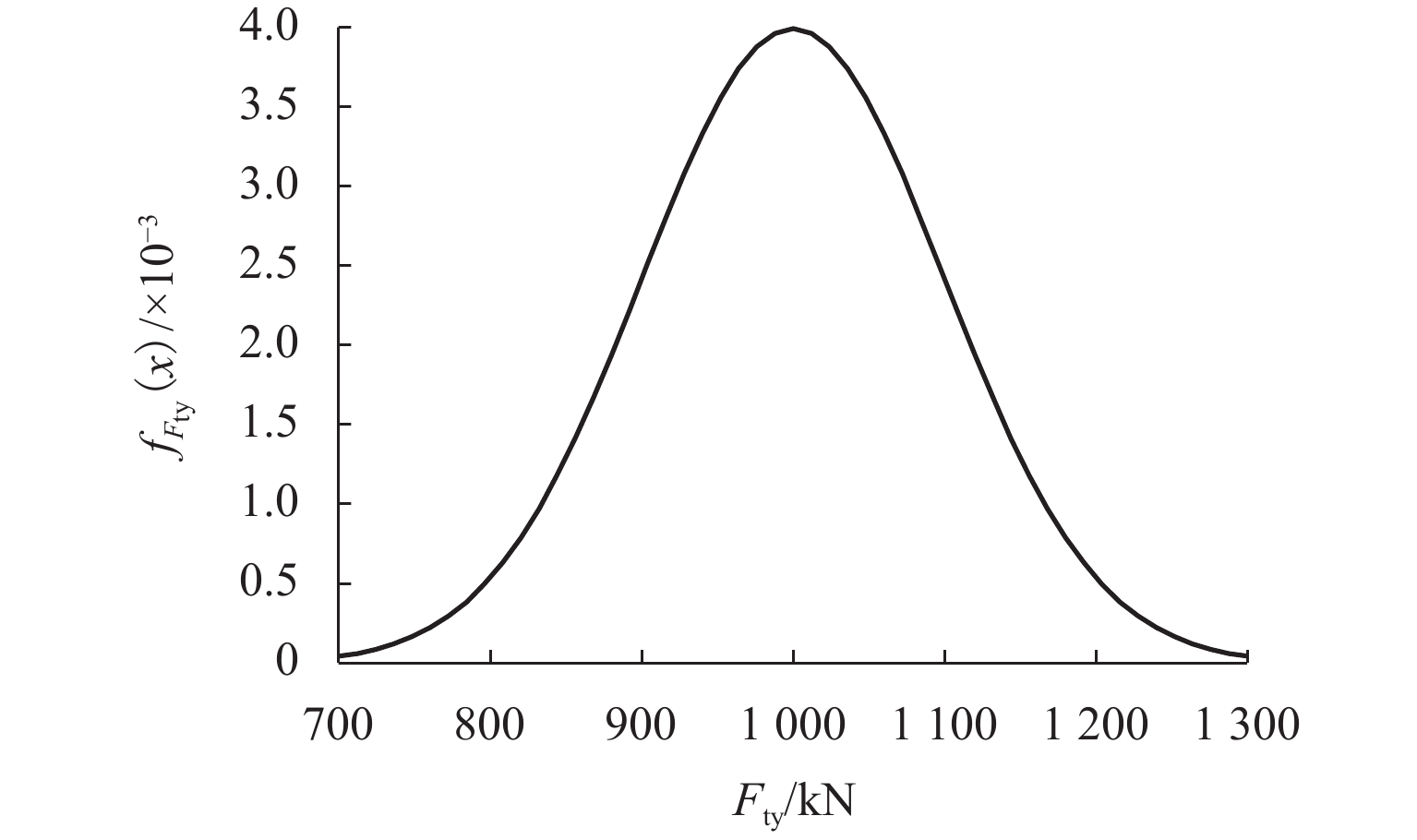
本文依据LS-FA-211001吸力锚的设计指标,并结合工程经验,假设锚链拉力的竖直分量{F_{{\mathrm{ty}}}}服从正态分布,且其均值{u_{\mathrm{S}}} = 1\;000,均方差{\sigma _{\mathrm{S}}} = 100,其对应的概率密度函数如图6所示.
从而,在吸力锚作用w次后,该型吸力锚的时变可靠度可以表示为
\begin{split} & R(w) = P({F_{{\mathrm{ty}}}}(w) < {T_{\mathrm{k}}}(w))= \\ &\quad \int_{ - \infty }^{ + \infty } {{f_{{T_{\mathrm{k}}}}}(x){{({F_{{F_{{\mathrm{ty}}}}}}(x))}^w}{\mathrm{d}}x} , \end{split} (11) 式中: {F_{{F_{{\mathrm{ty}}}}}}(x) 为锚链拉力竖直分量{F_{{\mathrm{ty}}}}的累积分布函数.
最终,利用给出的时变可靠性模型,针对LS-FA-211001吸力锚在不同勘探点(点A、C、G、M、O)处的抗拔力量化数据,进行时变可靠性分析,具体计算结果如图7所示.
由图7可知:在勘探点A处,吸力锚的可靠性最差,随着使用次数的增加,其可靠度下降,再次说明了在勘探点A处的吸力锚最容易失效. 然而,即使在恶劣的勘探点A处,在可靠度需达到95%的要求下,LS-FA-211001吸力锚仍然能够使用100次左右,说明了其具有较好的环境适应性. 而在其他勘探点处的良好环境下,该型吸力锚的寿命接近于无限使用次数,说明了LS-FA-211001吸力锚具有较高的可靠性. 本文的计算结果是基于一种简化的吸力锚受力分析展开的,与实际工程中的真实情况存在一定偏差. 同时,图7中的结果仅为实际工程中决策者对吸力锚的可靠性了解及制定相应的运行维护周期提供参考.
3. 模型验证
由LS-FA-211001吸力锚的时变可靠性分析结果可知,勘探点A处的吸力锚最容易失效,时变可靠度最低. 因此,本节主要对勘探点A处的时变可靠度结果开展验证分析,以说明本文所得吸力锚时变可靠度结果的有效性. MCS方法是一种经典的数理统计方法,由于其具有较高的估计精度,因而往往用于新模型的验证. MCS方法中用于新模型可靠性结果的验证误差为
\varepsilon = \frac{{\left| {P_{\mathrm{f}} - P_{{\mathrm{f_{MCS}}}}} \right|}}{{P_{\mathrm{{f_{MCS}}}}}} \times 100{\text{%}} , (12) 式中:P_{\mathrm{f}}为利用新模型评估出来的失效概率,P_{{\mathrm{f_{MCS}}}}为采用MCS方法评估出来的失效概率.
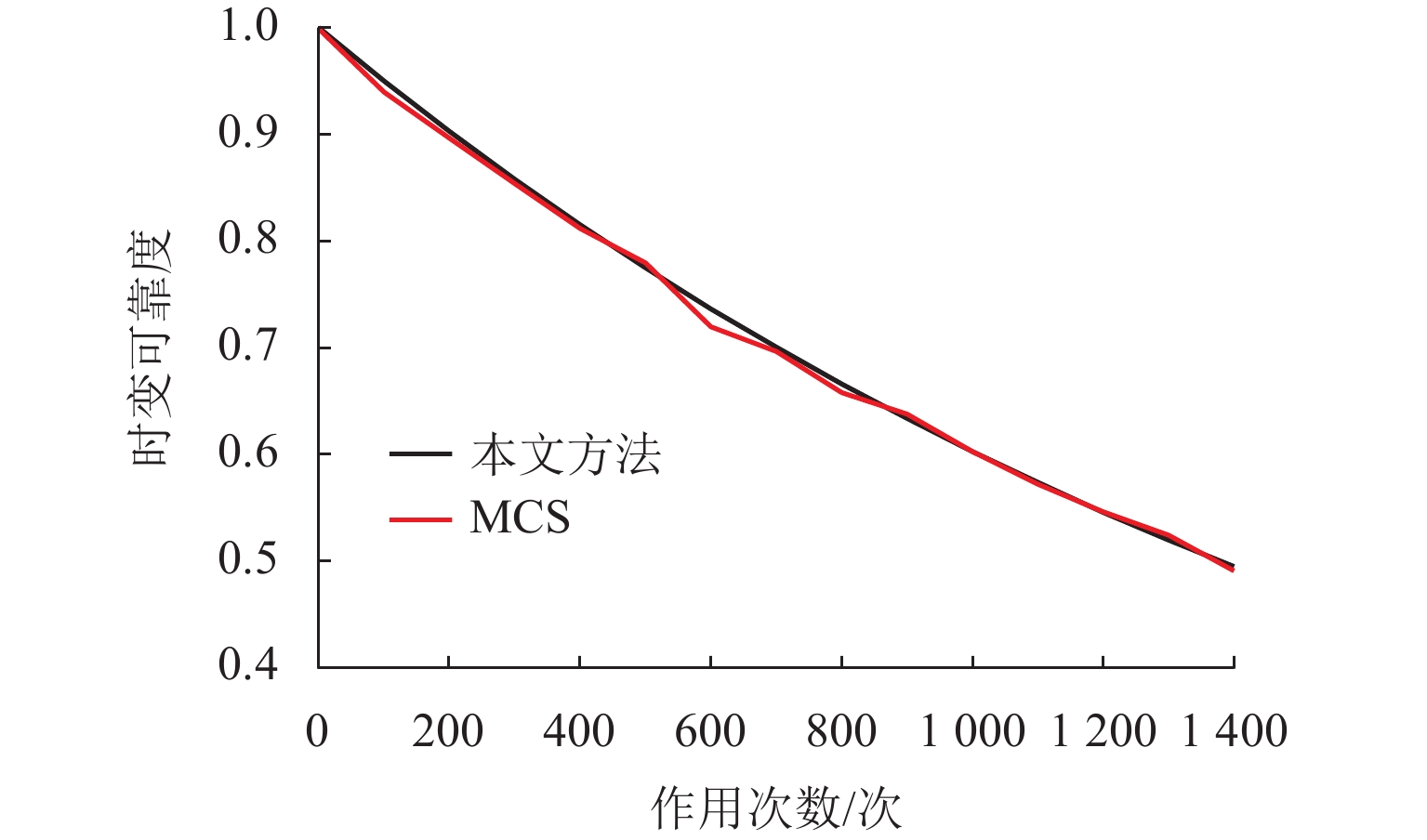
采用MCS方法计算吸力锚在勘探点A处的时变可靠度,并与本文方法所得结果进行对比,具体结果如图8所示.
由图8可知:利用本文给出的时变可靠性模型计算的勘探点A处可靠度结果与MCS方法计算出来的结果基本一致,验证了本文方法的有效性;MCS方法与本文给出的吸力锚时变可靠性模型的最大时变可靠度计算误差为2.15%,进一步说明了本文方法的准确性,可为工程实际中对吸力锚的可靠性研究提供一定指导.
4. 结 论
本文建立了循环载荷作用下吸力锚的时变可靠性模型,利用MATLAB软件编制了对应的程序代码. 同时,收集计算了LS-FA-211001吸力锚在不同勘探点的抗拔力,并进行了不确定性量化分析. 进一步建立了不同勘探点处吸力锚应力-强度干涉模型,进行了循环载荷作用下吸力锚的时变可靠性分析,得到主要结论如下:
1) 吸力锚在勘探点A的抗拔力相对较小,在其他勘探点处的抗拔力相对差异不大,说明勘探点A处的吸力锚是最容易失效的.
2) 即使在恶劣的勘探点A,可靠度95%以上的条件下,LS-FA-211001吸力锚仍然能够使用100次左右,而在其他勘探点处基本是无限使用次数,说明了该型吸力锚可靠性高.
3) 利用MCS方法对勘探点A处的时变可靠度结果进行验证,本文方法的最大验证误差为2.15%,满足精度要求,说明了本文方法的有效性.
本文所得结果是基于一种吸力锚简化的受力分析展开的,与实际工程存在一定偏差,仅为工程实际中吸力锚的前期设计提供指导,为工程决策者提供参考.
-
表 1 各勘探点附近的抗拔力值
Table 1. Pulling resistance values near each exploratory point
kN 组号 点A 点C 点G 点M 点O 1 1330.9777 2007.6882 2247.3961 1826.8866 1835.3776 2 1339.7254 1998.8052 2261.1515 1820.1196 1840.0185 3 1336.7282 1982.7419 2274.1373 1814.4576 1843.6222 4 1330.8861 1964.7617 2286.1875 1809.4007 1846.4546 5 1323.8135 1947.6050 2297.1344 1804.6813 1848.9262 6 1319.7456 1954.0500 2191.5069 1800.0756 1851.5450 7 1319.9079 1970.4304 2187.9786 1795.7828 1854.8203 8 1322.8437 1987.0357 2182.8988 1792.0530 1859.1009 9 1328.1747 2003.6953 2176.1155 1791.3837 1864.3240 10 1338.2259 2020.2961 2167.4843 1791.0338 1869.6512 表 2 各勘探点附近抗拔力分布参数
Table 2. Parameters of pulling resistance distribution near each exploratory point
勘探点 u \sigma A 1329.1029 7.4612 C 1983.7110 24.2016 G 2227.1991 48.0464 M 1804.5875 12.7894 O 1851.3841 10.7934 -
[1] 王胤,朱兴运,杨庆. 考虑砂土渗透性变化的吸力锚沉贯及土塞特性研究[J]. 岩土工程学报,2019,41(1): 184-190.WANG Yin, ZHU Xingyun, YANG Qing. Installation of suction caissons and formation of soil plug considering variation of permeability of sand[J]. Chinese Journal of Geotechnical Engineering, 2019, 41(1): 184-190. [2] 霍知亮. 黏土地基中桶形基础模型试验及工作机理研究[D]. 天津:天津大学,2015. [3] 王胤,杨涵,庞子毅,等. 基于CFD-DEM流固耦合方法的吸力锚基础负压沉贯数值模拟[J]. 岩土工程学报,2023,45(2): 384-393,444. doi: 10.11779/CJGE20211512WANG Yin, YANG Han, PANG Ziyi, et al. Numerical simulation of negative pressure penetration of suction anchor foundation based on CFD-DEM fluid solid coupling method[J]. Chinese Journal of Geotechnical Engineering, 2023, 45(2): 384-393,444. doi: 10.11779/CJGE20211512 [4] 闫宏生,苑恒,李怀亮,等. 大抓力锚和吸力锚在CALM型单点系泊系统中的应用[J]. 中国海洋平台,2022,37(4): 31-36,42.YAN Hongsheng, YUAN Heng, LI Huailiang, et al. Application of fluke anchor and suction anchor in CALM single-point mooring system[J]. China Offshore Platform, 2022, 37(4): 31-36,42. [5] RICE S O. Mathematical analysis of random noise[J]. The Bell System Technical Journal, 1944, 23(3): 282-332. doi: 10.1002/j.1538-7305.1944.tb00874.x [6] CRANDALL S H, CHANDIRAMANI K L, COOK R G. Some first-passage problems in random vibration[J]. Journal of Applied Mechanics, 1966, 33(3): 532-538. doi: 10.1115/1.3625118 [7] ANDRIEU-RENAUD C, SUDRET B, LEMAIRE M. The PHI2 method: a way to compute time-variant reliability[J]. Reliability Engineering & System Safety, 2004, 84(1): 75-86. [8] SINGH A, MOURELATOS Z P. On the time-dependent reliability of non-monotonic, non-repairable systems[J]. SAE International Journal of Materials and Manufacturing, 2010, 3(1): 425-444. doi: 10.4271/2010-01-0696 [9] HU Z, DU X P. A sampling approach to extreme value distribution for time-dependent reliability analysis[J]. Journal of Mechanical Design, 2013, 135(7): 071003.1-071003.8. [10] HU Z, DU X P. Time-dependent reliability analysis with joint upcrossing rates[J]. Structural and Multidisciplinary Optimization, 2013, 48(5): 893-907. doi: 10.1007/s00158-013-0937-2 [11] JIANG C, HUANG X P, HAN X, et al. A time-variant reliability analysis method based on stochastic process discretization[J]. Journal of Mechanical Design, 2014, 136(9): 091009.1-091009.11. [12] LI C Y, ZHANG Y M. Time-variant reliability assessment and its sensitivity analysis of cutting tool under invariant machining condition based on gamma process[J]. Mathematical Problems in Engineering, 2012, 2012: 676923.1-676923.19. [13] CAZUGUEL M, RENAUD C, COGNARD J Y. Time-variant reliability of nonlinear structures: application to a representative part of a plate floor[J]. Quality and Reliability Engineering International, 2006, 22(1): 101-118. doi: 10.1002/qre.750 [14] KOPUSTINSKAS V, AUGUTIS J, RIMKEVIČIUS S. Dynamic reliability and risk assessment of the accident localization system of the Ignalina NPP RBMK-1500 reactor[J]. Reliability Engineering & System Safety, 2005, 87(1): 77-87. [15] 张德权,韩旭,姜潮,等. 时变可靠性的区间PHI2分析方法[J]. 中国科学:物理学 力学 天文学,2015,45(5):41-53.ZHANG D Q, HAN X, JIANG C, et al. The interval PHI2 analysis method for time-dependent reliability[J]. Sci Sin-Phys Mech Astron, 2015, 45(5):41-53. [16] TIAN L, NOORE A. Dynamic software reliability prediction: an approach based on support vector machines[J]. International Journal of Reliability, Quality and Safety Engineering, 2005, 12: 309-321. doi: 10.1142/S0218539305001847 [17] BECKER G, CAMARINOPOULOS L, KABRANIS D. Dynamic reliability under random shocks[J]. Reliability Engineering & System Safety, 2002, 77(3): 239-251. [18] STREICHER H, RACKWITZ R. Time-variant reliability-oriented structural optimization and a renewal model for life-cycle costing[J]. Probabilistic Engineering Mechanics, 2004, 19(1/2): 171-183. [19] SUDRET B. Analytical derivation of the outcrossing rate in time-variant reliability problems[J]. Structure and Infrastructure Engineering, 2008, 4(5): 353-362. doi: 10.1080/15732470701270058 [20] 茆诗松,王静龙,濮晓龙. 高等数理统计[M]. 2版. 北京:高等教育出版社,2006:34-35. [21] 钟泽棋. 基于FMECA的吸力锚系统可靠性评估[D]. 成都:电子科技大学,2023. -






 下载:
下载:







 下载:
下载: