考虑黏结-滑移效应的UHPC梁钢筋应力计算方法

孙永新; 蔺鹏臻; 杨子江

doi:10.3969/j.issn.0258-2724.20230130

考虑黏结-滑移效应的UHPC梁钢筋应力计算方法

doi: 10.3969/j.issn.0258-2724.20230130

兰州交通大学土木工程学院，甘肃兰州 730070

基金项目: 国家自然科学基金项目（U1934205）

详细信息

作者简介:
孙永新（1989—），男，讲师，博士研究生，研究方向为UHPC桥梁结构设计理论与工程应用，E-mail：syx170007@163.com

通讯作者:
蔺鹏臻（1977—），男，教授，博士生导师，研究方向为大跨度桥梁设计理论及建造技术，E-mail：pzhlin@mail.lzjtu.cn

中图分类号: U443.32
计量
- 文章访问数: 275
- HTML全文浏览量: 113
- PDF下载量: 50
- 被引次数: 0
出版历程
- 收稿日期: 2023-03-26
- 修回日期: 2023-07-13
- 网络出版日期: 2024-06-17
- 刊出日期: 2023-10-30

Calculation Method for Reinforcement Stress in Ultra-High Performance Concrete Beams Considering Bond-Slip Effect

School of Civil Engineering, Lanzhou Jiaotong University, Lanzhou 730070, China

摘要

摘要:
为建立适用于配筋超高性能混凝土（UHPC）梁的钢筋应力计算方法，对6片UHPC-T形截面梁开展四点弯曲试验，研究钢筋应力的变化规律. 从钢筋-UHPC受力平衡与变形协调机理出发，应用微元体建立平衡、变形以及黏结-滑移微分方程，导出能综合反映钢筋与UHPC界面黏结-滑移影响及钢纤维抗拉贡献的钢筋应力计算公式，并通过简化应变不均匀系数与裂缝截面钢筋应力计算，提出便于工程应用的钢筋应力简化公式. 研究表明：单位荷载下钢筋应力的增幅随配筋率的提高而减小，而与钢纤维体积率的变化无关；与普通混凝土梁相比，UHPC梁的钢筋应力在开裂截面处偏小，但其分布在相邻裂缝间的不均匀程度更高；钢筋应力建议公式计算值与本文、既有文献的试验值均吻合良好；钢筋应力简化公式计算值与试验值之比的均值为1.03，变异系数为0.06，表明该简化式可用于UHPC梁的钢筋应力计算.
- 超高性能混凝土（UHPC） /
- 钢筋应力 /
- 黏结-滑移 /
- 应变不均匀系数 /
- 微元体
Abstract:
Four-point bending tests were conducted on six ultra-high performance concrete T-shaped (UHPC-T) section beams to establish a reinforcement stress calculation method for reinforced UHPC beams and study the variation law of reinforcement stress. Based on the mechanism of force balance and deformation coordination between reinforcement and UHPC, a reinforcement stress calculation formula was derived using the differential equations of equilibrium, deformation, and bond-slip established by micro elements, which could comprehensively reflect the influence of bond-slip between reinforcement and UHPC interfaces and the contribution of steel fibers to tensile strength. By simplifying the calculation of the strain non-uniformity coefficient and the reinforcement stress in cracked sections, a simplified formula for reinforcement stress suitable for engineering applications was proposed. The results show that the increase in reinforcement stress under unit load decreases with the increase in reinforcement ratio, but it is not related to the change in steel fiber volume fraction. Compared with ordinary concrete beams, the reinforcement stress in UHPC beams is relatively small in the cracked section, but the uneven distribution of reinforcement stress between adjacent cracks is intensified. The calculation value of the suggested formula for reinforcement stress is in good agreement with the experimental values in this article and existing literature. The average ratio of the calculated value of the simplified formula for reinforcement stress to the experimental value is 1.03, and the coefficient of variation is 0.06, indicating that this simplified formula can be used for calculating reinforcement stress in UHPC beams.
- ultra-high performance concrete (UHPC) /
- reinforcement stress /
- bond-slip /
- strain non-uniformity coefficient /
- micro elements

HTML全文

超高性能混凝土（UHPC）是一种具有超高强度、高断裂韧性和高耐久性等优良性能的新型水泥基复合材料，能有效提高结构抗裂能力，延长结构服役年限^[1-3]. 在正常使用极限状态下，UHPC基本结构的力学性能研究是UHPC在桥梁工程广泛应用的前提之一. 其中，裂缝宽度是评估桥梁结构在正常使用极限状态验算的重要指标，而纵向受拉钢筋应力是影响裂缝宽度计算的首要因素.

目前，国内学者建议的UHPC梁钢筋应力计算方法主要考虑了钢纤维抗拉贡献，采用试验数据修正了《混凝土结构设计规范》（GB 50010—2010）^[4]的钢筋应力计算公式^[5-7]. 在该规范中，影响裂缝宽度的首要因素是相邻裂缝间钢筋的平均应力，其值为钢筋应变不均匀系数与裂缝截面钢筋应力的乘积，而文献[7]尽管修正了两参数，但忽视了钢筋平均应力的验证. 另外，钢筋平均应力计算的理论基础是相邻裂缝间钢筋应力的分布模式^[8]，规范GB 50010—2010基于大量试验结果，采用半理论半经验法给出了钢筋平均应力计算模式，而UHPC结构致密，且基体内存在钢纤维，更强的界面黏结应力使得钢筋应力在相邻裂缝间的分布不均匀程度发生变化^[9]. 同时，其优异的抗拉强度、韧度等特性及钢纤维的抗拉贡献使得UHPC能够有效分担部分应力^[10-11]，进而使钢筋应力减小. 因此，直接通过试验数据修正规范公式从而实现普通混凝土与UHPC的转换缺乏理论依据. 此外，国外学者主要通过UHPC梁的四点抗弯试验，研究钢筋应变与试验荷载的关系以及钢筋应变的变化规律. 文献[12-14]基于配筋UHPC梁的抗弯试验，分别研究普通钢筋、GFRP筋的应变与试验荷载的关系，分析钢筋应变在加载过程中的变化规律及配筋率、UHPC强度等因素对配筋应变的影响，但对UHPC梁钢筋应力的理论计算方法却鲜有研究. 因此，有必要结合UHPC梁的试验结果，建立适用于UHPC梁的钢筋应力计算公式.

本文首先以钢纤维体积率、配筋率为参变量，对6片UHPC-T形截面梁开展四点抗弯试验，分析钢筋应力的变化规律；其次，充分考虑钢筋与UHPC界面黏结-滑移特性、钢纤维抗拉贡献对钢筋应力的影响，运用微元体建立平衡、相容以及黏结-滑移微分方程，求解得到UHPC梁的钢筋应力计算公式；最后，为便于工程化应用，简化钢筋应变不均匀系数与裂缝截面钢筋应力，给出适用于UHPC梁的钢筋应力简化公式.

1. 试验研究

1.1 试验设计及加载方案

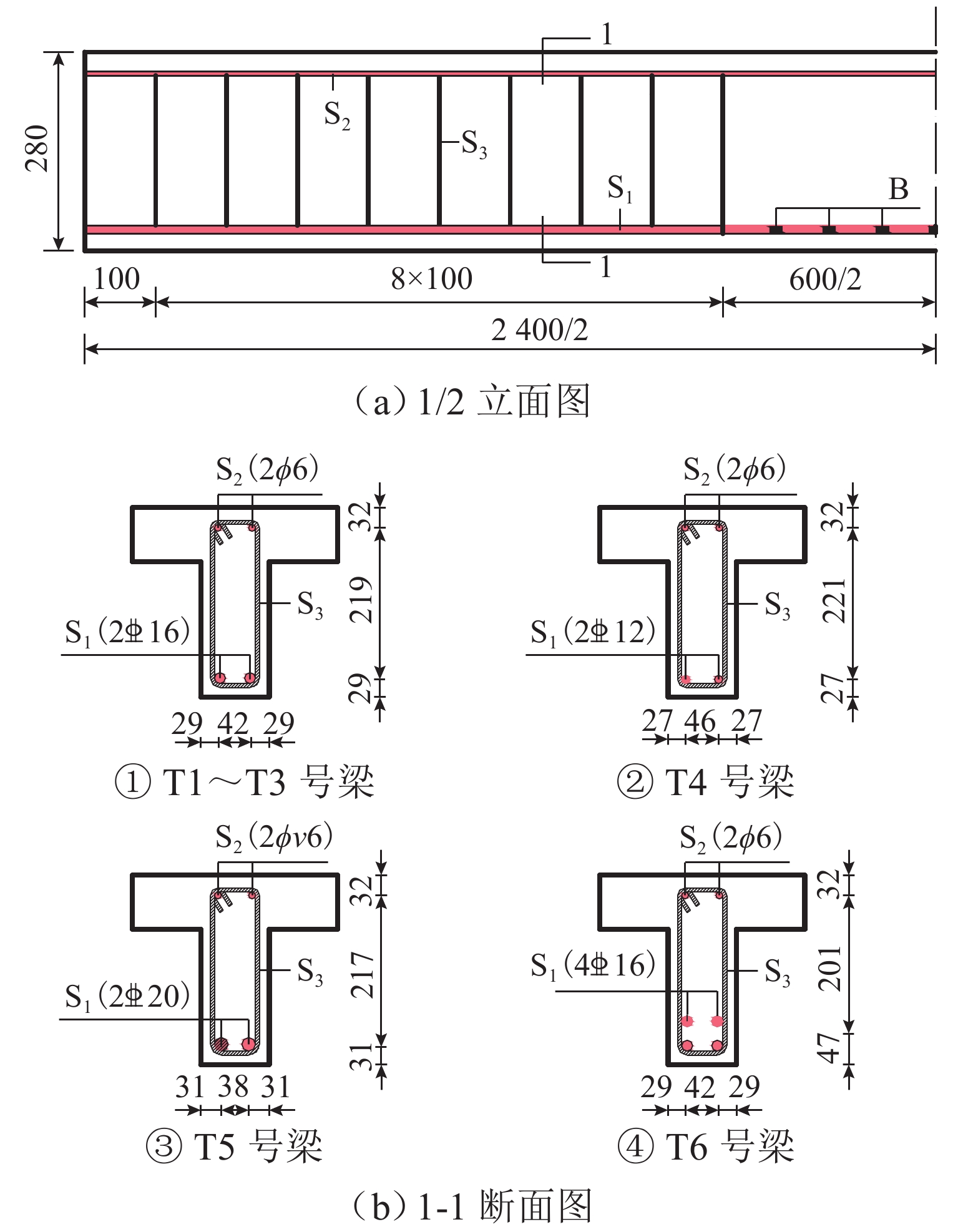
共设计了6片UHPC-T形截面梁试件，每片试件长2400 mm，净跨长2200 mm，纯弯段长600 mm，试件变化参数如表1所示，配筋情况及截面尺寸如图1所示. 表1中：V_f、ρ_s和c分别为钢纤维体积率、配筋率和保护层厚度. 图1中：S₁、S₂、S₃和B分别为纵向受拉钢筋、受压钢筋、箍筋和粘贴在S₁上的电阻应变片，其中，S₁采用HRB400级钢筋，S₂、S₃均采用直径为6 mm的光滑圆钢筋.

表 1 试件的编号与参数

Table 1. Number and parameters of specimens

变量	梁号	V_f/%	纵筋配置	ρ_s/%	c/mm
标准梁	T1	2	216	1.60	15
钢纤维体积率	T2	1	216	1.60
钢纤维体积率	T3	3	216	1.60
配筋率	T4	2	212	0.89
	T5		220	2.51
	T6		416	3.20

下载: 导出CSV

| 显示表格

图 1 梁的配筋及截面尺寸

Figure 1. Reinforcement and section dimensions of specimens

下载: 全尺寸图片幻灯片

制作UHPC的原材料包括水泥、矿粉、硅灰、细砂、高效聚羧酸减水剂和钢纤维等，其中水泥、矿粉、硅灰、细砂、高效聚羧酸减水剂的质量比为1.000∶0.150∶0.250∶1.200∶0.028，水胶比为0.18. 钢纤维采用平均长度为13 mm、长径比为61的平直型钢纤维. 参照《活性粉末混凝土》（GB/T 31387—2015）^[15]所述方法测定UHPC基本力学指标，其平均值见表2. 表中：f_cu为立方体抗压强度， f_c为棱柱体轴心抗压强度， f_t为轴心抗拉强度，E_c 为UHPC的弹性模量.

表 2 基本力学指标

Table 2. Basic mechanical indicators

V_f/%	f_cu/MPa	f_c/MPa	f_t/MPa	E_c/GPa
1	121.22	83.72	6.35	41
2	133.71	89.14	7.84	42
3	141.53	97.82	9.32	44

下载: 导出CSV

| 显示表格

四点抗弯试验在液压试验机上进行，加载装置如图2（a）所示. 加载采用分级制，每级荷载加载值及加载时间参照《混凝土结构试验方法标准》（GB/T 50152—2012）^[16]规定. 加载初期每级荷载加载值为10 kN；加载值达到预估开裂荷载值的80%后，每级加载值调整为5 kN；试件开裂后，每级加载值再调整为10 kN，并将每级荷载持续时间设定为15 min；荷载达到预估钢筋屈服荷载的80%后，每级加载值再次调整为5 kN；加载值接近预估极限试验荷载值时，放缓加载速度，直至梁体破坏.

图 2 四点抗弯试验

Figure 2. Four-point bending test

下载: 全尺寸图片幻灯片

为有效采集钢筋应变数据，在试件纯弯段每根受拉钢筋等间距粘贴7个应变片（如图1（a））. 钢筋应变由INV2366静态数据采集仪采集，频率设定为1次/5 s，数据采集系统如图2（b）所示. 观测的裂缝间距是指梁试件纯弯段内相邻有效可视裂缝（裂缝宽度不低于0.05 mm）的间距，由毫米直尺测量，平均裂缝间距取有效裂缝间距的均值.

1.2 钢筋应力的发展过程及变化规律分析

1.2.1 钢筋应力的发展过程

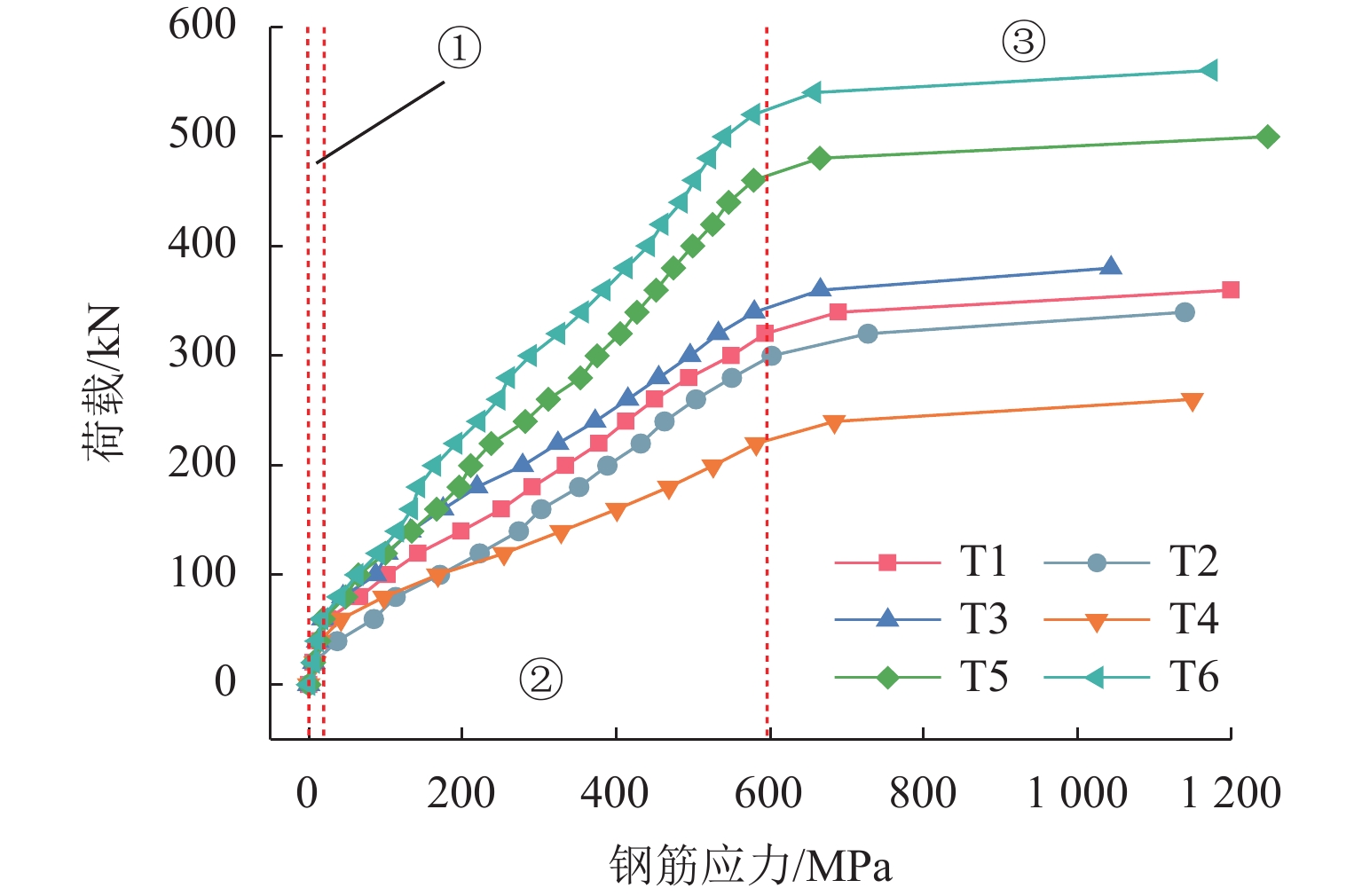
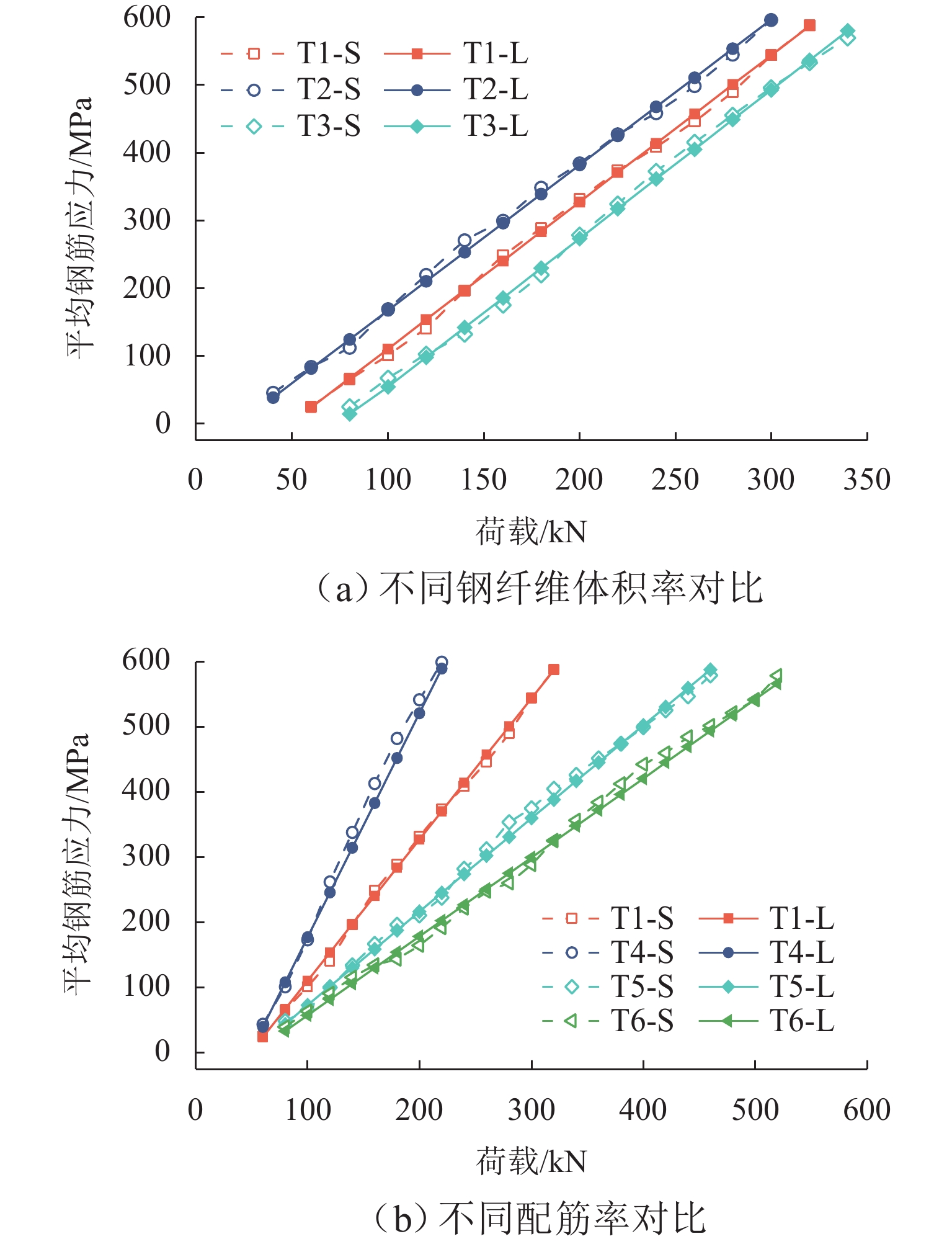
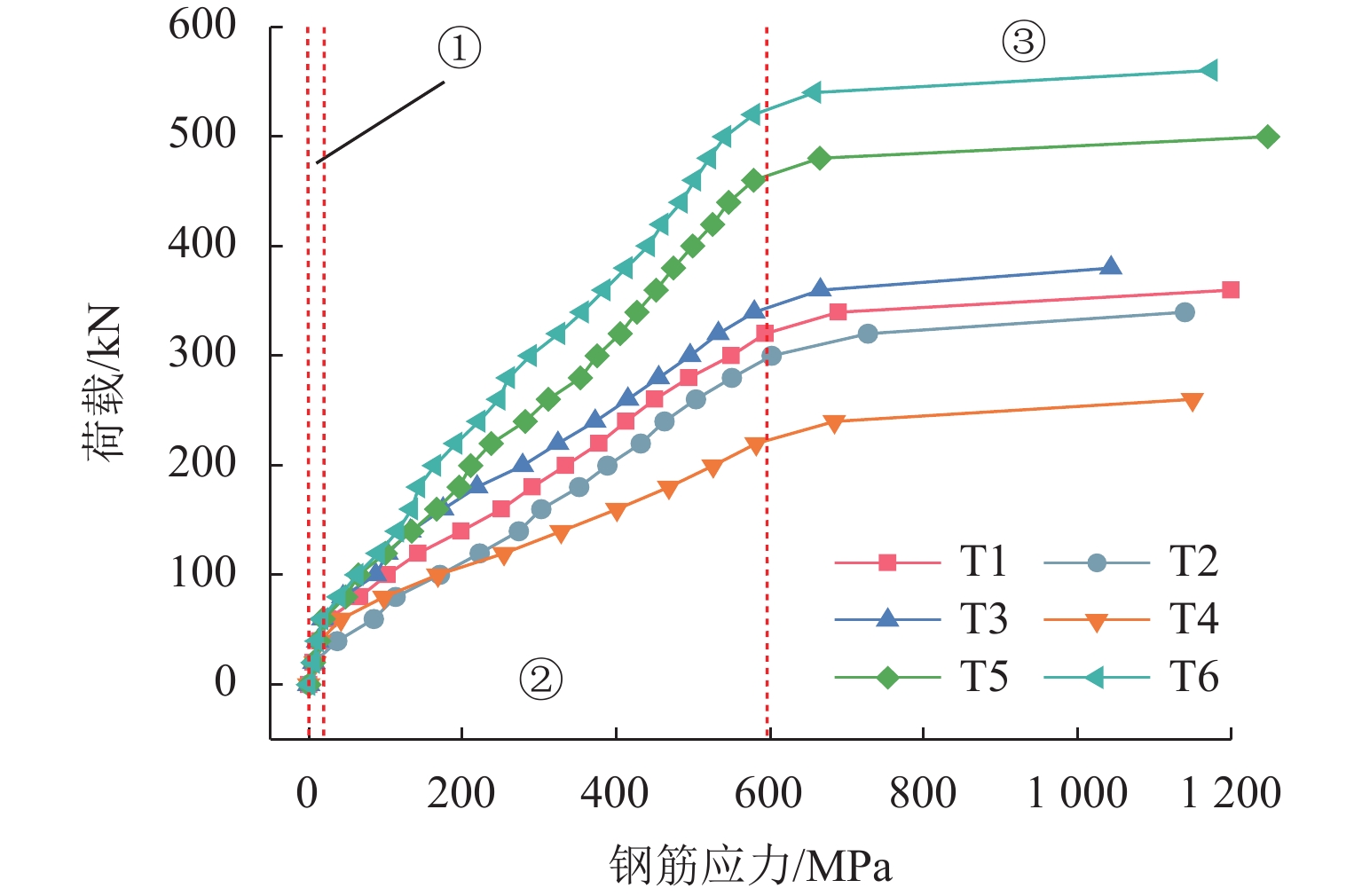
有效钢筋应力实测值的均值与试验荷载关系曲线如图3所示，根据该曲线可将钢筋应力的发展过程分为线弹性阶段（①）、裂缝发展阶段（②）和屈服强化阶段（③）.

图 3 荷载-钢筋应力关系曲线

Figure 3. Load-reinforcement stress relationship curves

下载: 全尺寸图片幻灯片

在线弹性阶段，试验荷载小于开裂荷载，试件表面未出现裂缝，钢筋应力与试验荷载呈线性关系. 当试验荷载达到开裂荷载时，在试件底部薄弱区首先出现1～2条微裂缝，曲线斜率发生偏离，试件进入裂缝发展阶段. 该阶段裂缝数量逐渐增多，裂缝宽度缓慢且稳定地开展，钢纤维开始发挥桥接作用，钢筋应力与荷载近似呈线性关系，与线弹性阶段相比，曲线斜率明显减小. 随着荷载继续增大，曲线斜率再次发生偏离，试件进入屈服强化阶段. 该阶段试验荷载变化较小，裂缝数量和间距趋于稳定，但裂缝宽度不断扩大，并出现1～2条主裂缝，伴随钢纤维渐渐被拔出，钢筋应力迅速增加，最终受拉钢筋屈服，梁体破坏.

1.2.2 钢筋应力变化规律分析

本文重点关注梁试件在裂缝发展阶段的钢筋应力变化规律. 将图3中的开裂荷载F_cr、屈服荷载F_t、开裂钢筋应力σ_cr、屈服钢筋应力σ_t以及钢筋应力在裂缝发展阶段的增长率V_m列入表3. 表中：V_m为单位荷载下钢筋应力的增幅，即V_m=(σ_t−σ_cr)/(F_t−F_cr).

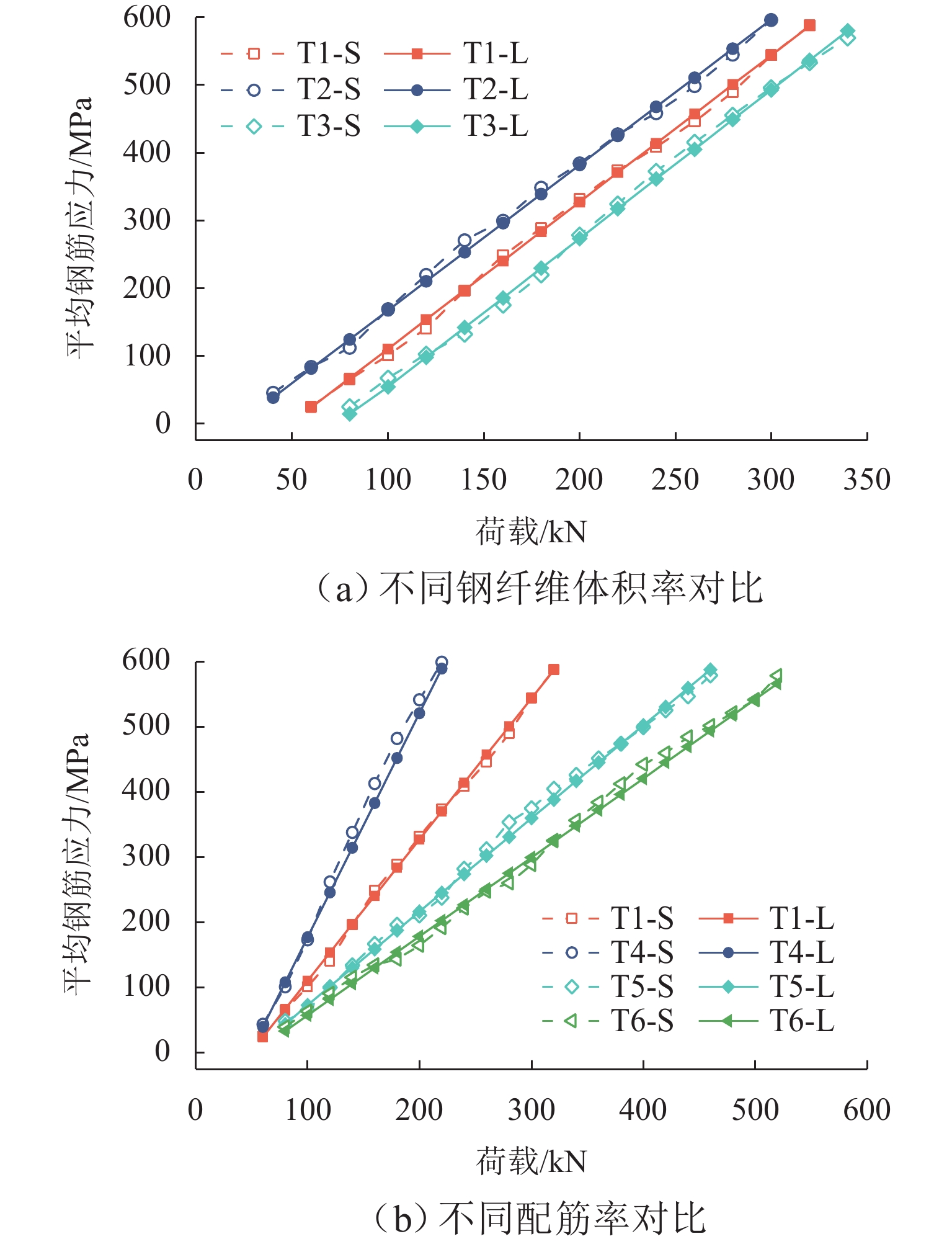
表 3 钢筋应力的实测结果

Table 3. Measured results of reinforcement stress

梁号	F_cr/kN	F_t/kN	σ_cr/MPa	σ_t/MPa	V_m/（MPa•kN⁻¹）
T1	55.24	321.42	37.77	593.75	2.09
T2	37.27	311.31	32.23	602.22	2.08
T3	69.72	334.27	44.53	579.63	2.02
T4	43.43	218.51	41.82	581.70	3.08
T5	60.67	454.88	48.34	579.06	1.35
T6	61.14	529.20	39.25	578.51	1.15

下载: 导出CSV

| 显示表格

综合图3、表3可知：钢纤维体积率从1%依次提高至2%、3%时，同级别荷载作用下的钢筋应力减小，但V_m基本一致，说明随着钢纤维体积率的提高，钢纤维承担的应力增加，钢筋应力减小，但不改变单位荷载下钢筋应力的增幅；配筋率从0.89%依次提高至1.60%、2.51%和3.20%时，同级别荷载作用下的钢筋应力与V_m均减小，说明钢筋应力及单位荷载下钢筋应力的增幅均随配筋率的提高而减小.

2. UHPC梁的钢筋应力计算

2.1 相邻裂缝间钢筋应力计算

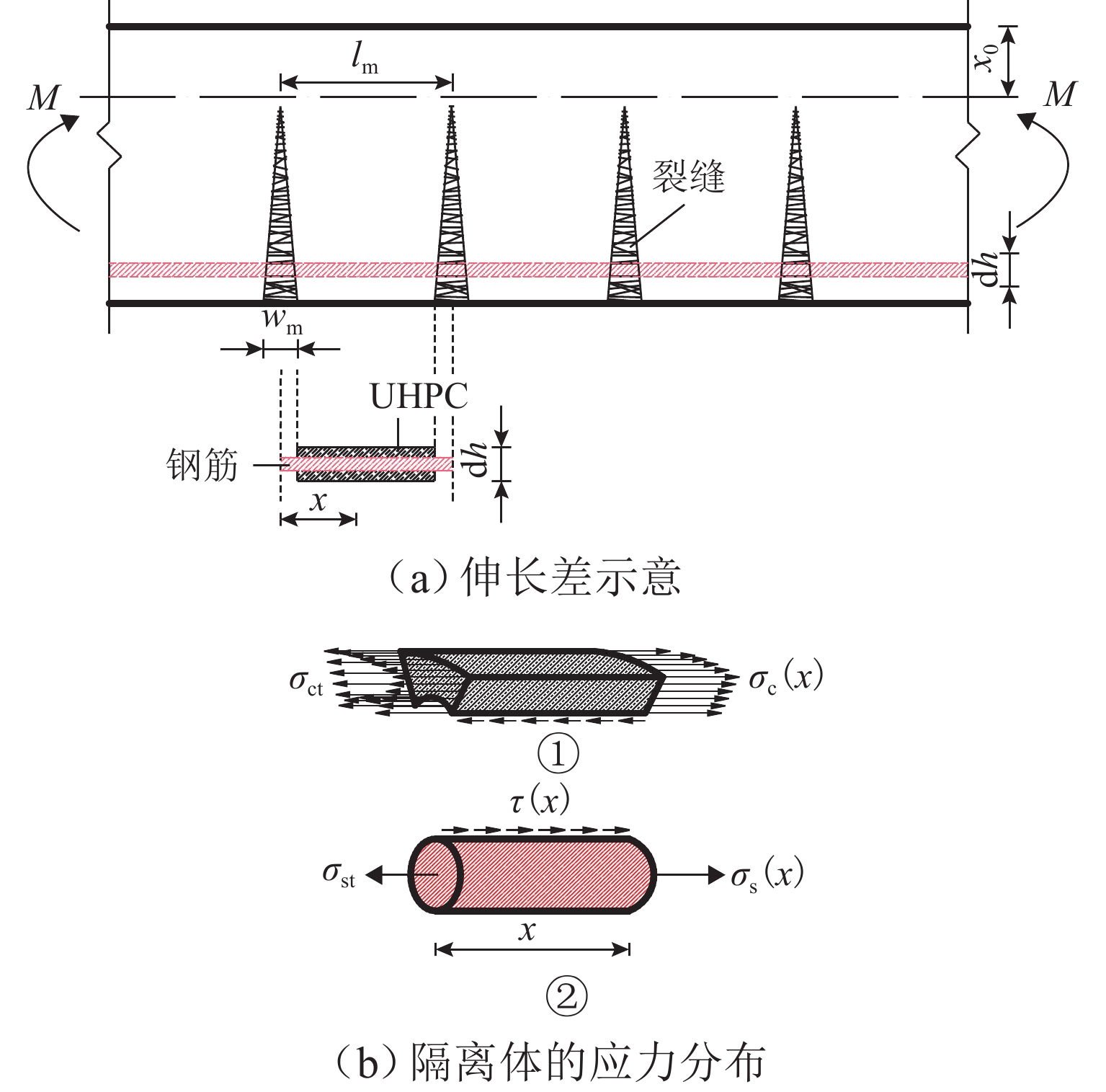
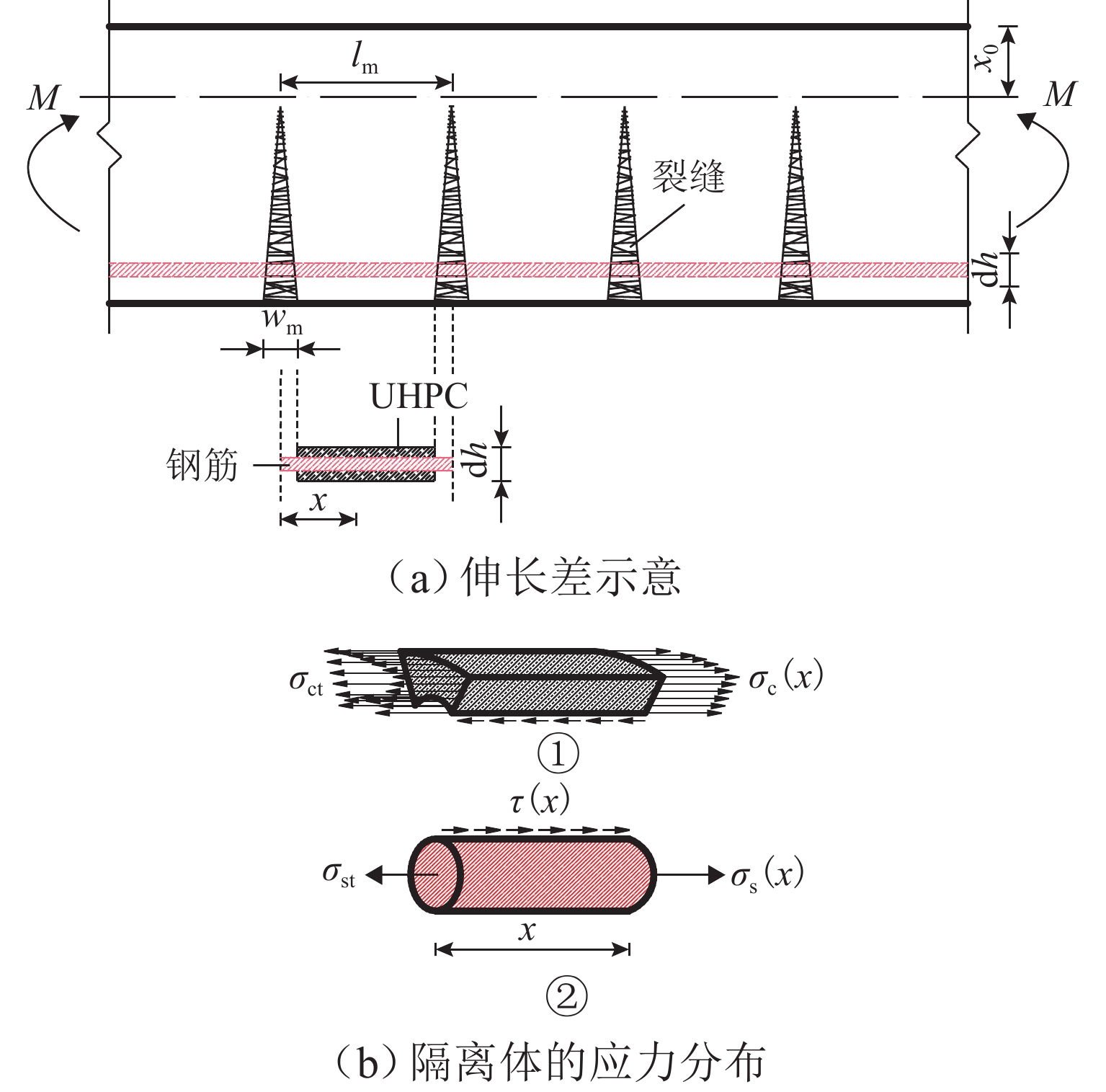
本文重点研究UHPC梁在裂缝发展阶段的钢筋应力计算方法. 图4为理想UHPC梁的变形及受力示意，取图中距离裂缝截面长度为x的隔离体（图4（b）），分析UHPC与钢筋之间的受力及变形协调机理. 图中：x₀为受压区高度，w_m、l_m分别为平均裂缝宽度与间距，σ_s(x)为钢筋在距离裂缝截面长度为x的截面拉应力，σ_c(x)为UHPC拉应力，τ(x)为钢筋与UHPC界面黏结应力，σ_st、σ_ct分别为钢筋和钢纤维在裂缝截面的拉应力，M为外荷载作用下产生的弯矩，dh为图4（a）隔离体的高度.

图 4 受弯构件开裂后的变形及受力

Figure 4. Deformation and stress of bending member after cracking

下载: 全尺寸图片幻灯片

根据图4（b）隔离体的应力分布，可将钢筋-UHPC视为组合配筋方式，UHPC与钢筋协同工作：在裂缝截面，基体退出工作，应力由钢纤维与钢筋共同承担，产生钢筋应力σ_st与钢纤维拉应力σ_ct；在未开裂截面，基体仍参与工作，钢筋与UHPC界面产生τ（x），且部分钢筋应力通过界面黏结应力传递给UHPC，产生σ_c(x).

由于钢筋与UHPC的受力机理及应力-应变关系不同，两者必然发生伸长差，产生裂缝，其裂缝宽度^[17] 为

${w_{\mathrm{m}}}{\mathrm{ = }}\left( {{\varepsilon _{{\mathrm{sm}}}} - {\varepsilon _{{\mathrm{cm}}}}} \right){l_{\mathrm{m}}}{\mathrm{ = }}{c_{\mathrm{c}}}\varphi {\mathrm{ }}{\sigma _{{\mathrm{st}}}}{l_{\mathrm{m}}}/{E_{\mathrm{s}}}{\mathrm{ = }}{c_{\mathrm{c}}}{\sigma _{{\mathrm{sm}}}}{l_{\mathrm{m}}}/{E_{\mathrm{s}}} \text{，}$

(1)

式中：$\varphi $为钢筋应变不均匀系数，σ_sm为钢筋在相邻裂缝间的平均应力，ε_sm、ε_cm分别为钢筋、UHPC在相邻裂缝间的平均应变，c_c为UHPC影响系数，E_s为纵向受拉钢筋的弹性模量.

由式（1）可知，σ_sm是影响裂缝宽度计算的首要因素，其理论计算式为

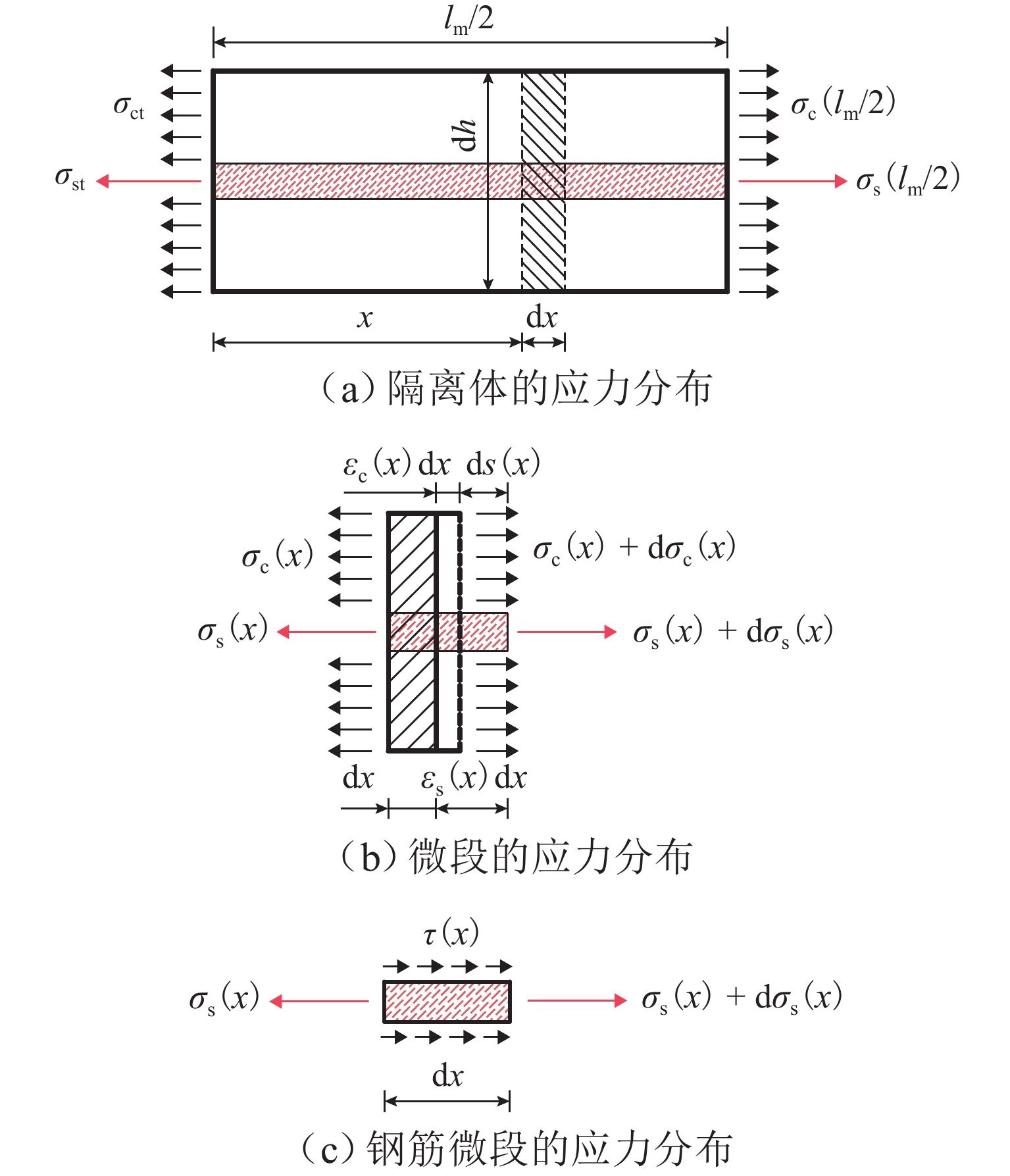
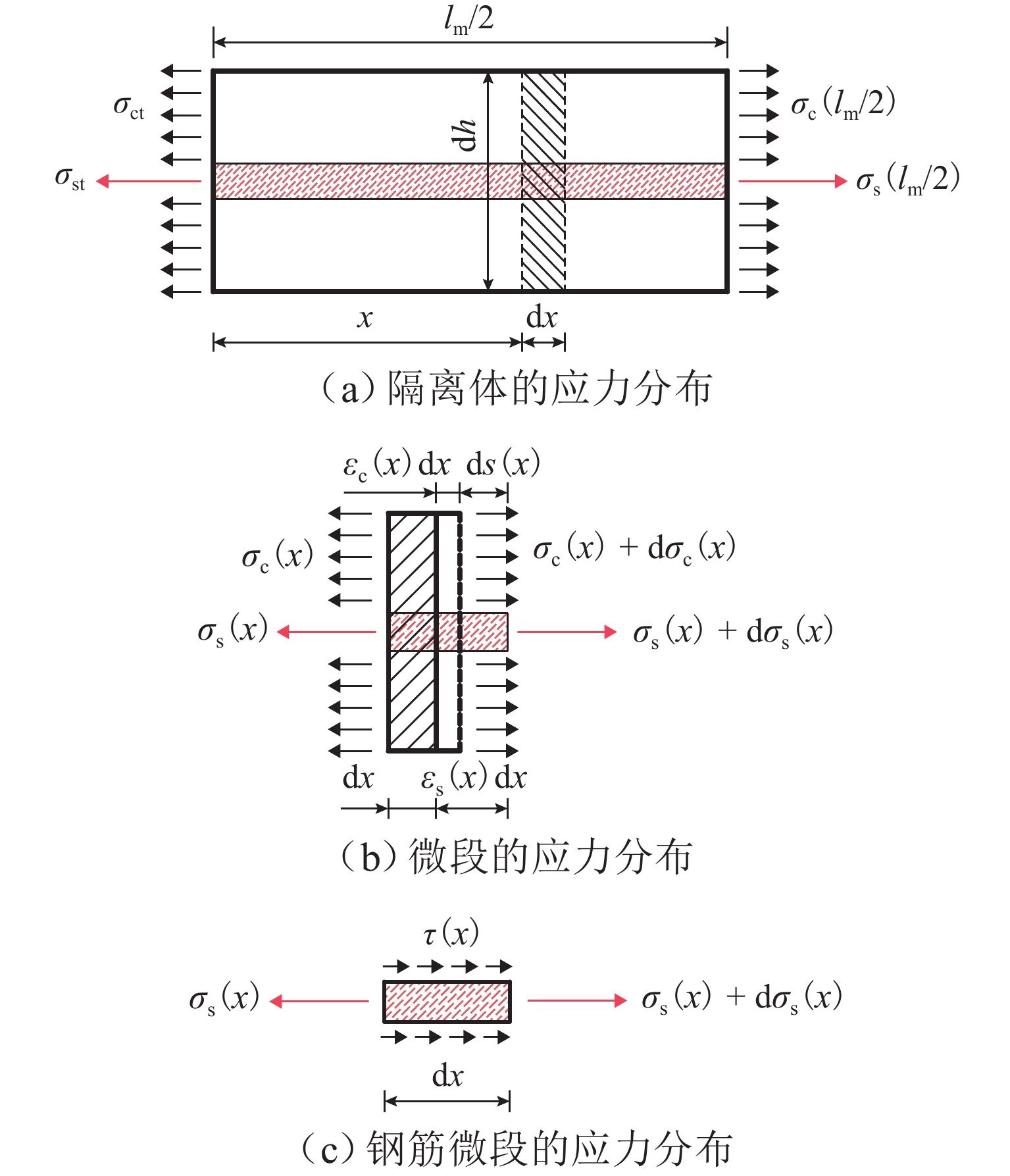
${\sigma _{{\text{sm}}}} = \int_0^{{l_{\text{m}}}} {{\sigma _{\text{s}}}(x)} {l_{\text{m}}}/{\text{d}}x.$

(2)

由式（2）可知，钢筋平均应力计算的理论基础为钢筋应力在相邻裂缝间的分布模式. 取图4裂缝区段内dh段部分为隔离体，建立图5所示的计算模型. 该隔离体可视为轴拉构件^[18]，构件中产生的应力在x=l_m/2处对称，应力分布如图5（a）所示. 隔离体微段（dx部分）的应力分布及变形示意如图5（b），钢筋微段的应力分布如图5（c）所示. 图中：ε_s(x)为钢筋在距离裂缝截面长度为x的截面拉应变，ε_c(x)为UHPC在距离裂缝截面长度为x的截面拉应变，s(x)为钢筋与UHPC在距离裂缝截面长度为x的截面位移差.

图 5 隔离体及微段的应力分布

Figure 5. Stress distribution of isolator and micro-segment

下载: 全尺寸图片幻灯片

根据图5（b）、（c）可知，微元体应满足应力平衡、变形相容方程，如式（3）～（5）.

$\tau \left( x \right){\text{ + }}\frac{{{A_{\text{s}}}}}{{{u_{\text{s}}}}}\frac{{{\text{d}}{\sigma _{\text{s}}}\left( x \right)}}{{{\text{d}}x}}{\text{ = }}0 ,$

(3)

${A_{\text{s}}}{\text{d}}{\sigma _{\text{s}}}\left( x \right){\text{ + }}{A_{\text{c}}}{\text{d}}{\sigma _{\text{c}}}\left( x \right){\text{ = }}0 ,$

(4)

${\text{d}}s\left( x \right) = \left( {{\varepsilon _{\text{s}}}\left( x \right) - {\varepsilon _{\text{c}}}\left( x \right)} \right){\text{d}}x ,$

(5)

式（3）～（5）中：$A_{\mathrm{s}} $、u_s分别为纵向受拉钢筋的截面面积、周长，$A_{\mathrm{c}} $为UHPC受拉区的有效面积.

在式（3）～（5）中包含σ_s(x)、σ_c(x)、τ(x)以及s(x) 4个未知量，方程数小于未知量个数，仍需建立一个方程才能求解. 式（5）反映了钢筋与UHPC之间的滑移关系，但在实际构件中，黏结与滑移是共生的，即黏结与滑移同时存在^[19]. 因此，可通过建立UHPC梁的黏结-滑移微分方程，联合式（3）～（5）进行求解. 文献[20]表明，UHPC梁在裂缝发展阶段的黏结-滑移微分方程为

$\frac{{{\text{d}}\tau \left( x \right)}}{{{\text{d}}x}} = k\frac{{{\text{d}}s\left( x \right)}}{{{\text{d}}x}},$

(6)

式中：k为滑移模量，与钢纤维体积率、配筋率及保护层厚度等因素有关^[10].

钢筋应力在相邻裂缝间的σ_s(x)通过联立式（3）～（6）求解得到. 将式（5）代入式（6）可得

$\frac{{{\text{d}}\tau \left( x \right)}}{{{\text{d}}x}} = k\left( \frac{{{\sigma _{\text{s}}}\left( x \right)}}{{{E_{\text{s}}}}} -\frac{{{\sigma _{\text{c}}}\left( x \right)}}{{{E_{\text{c}}}}} \right) .$

(7)

对式（4）两边求积分后代入式（7），得

$\frac{{{\text{d}}\tau \left( x \right)}}{{{\text{d}}x}} = k\left( {\frac{1}{{{E_{\text{s}}}}} + \frac{{{A_{\text{s}}}}}{{{E_{\text{c}}}{A_{\text{c}}}}}} \right){\sigma _{\text{s}}}\left( x \right) - \frac{k}{{{E_{\text{c}}}{A_{\text{c}}}}}\left( {{A_{\text{s}}}{\sigma _{{\text{st}}}} + {A_{\text{c}}}{\sigma _{{\text{ct}}}}} \right).$

(8)

对式（3）两边求一阶导数，得

$\frac{{{\text{d}}\tau \left( x \right)}}{{{\text{d}}x}} + \frac{{{A_{\text{s}}}}}{{{u_{\text{s}}}}}\frac{{{{\text{d}}^2}{\sigma _{\text{s}}}\left( x \right)}}{{{\text{d}}{x^2}}} = 0 .$

(9)

将式（8）代入式（9），得σ_s（x）的二阶微分方程为

$\frac{{{{\text{d}}^2}{\sigma _{\text{s}}}\left( x \right)}}{{{\text{d}}{x^2}}}{\text{ + }}k_1^2{\sigma _{\text{s}}}\left( x \right) = k_2^2,$

(10)

式中：$ k_1^2 = \dfrac{{k{u_{\text{s}}}}}{{{{{E}}_{\text{c}}}{A_{\text{c}}}}}\left( {1 + \dfrac{1}{{{\alpha _{\mathrm{E}}}{\rho _{{\text{te}}}}}}} \right) $，$ k_2^2 = \dfrac{{k{u_{\text{s}}}}}{{{E_{\text{c}}}{A_{\text{c}}}}}\left( {{\sigma _{{\text{sc}}}} + \dfrac{{{\sigma _{{\text{cc}}}}}}{{{\rho _{{\text{te}}}}}}} \right) $，α_E为钢筋与UHPC弹性模量的换算系数，ρ_te为有效配筋率.

根据式（10），可得

$\sigma_{\text{s}}\left(x\right)=\left(\sigma_{\text{st}}-\kappa\right)\cos\ k_1x+\kappa,$

(11)

式中：$ \kappa = {{k_2^2} \mathord{\left/ {\vphantom {{k_2^2} {k_1^2}}} \right. } {k_1^2}} = {\alpha _{\mathrm{E}}}{{\left( {{\rho _{{\text{te}}}}{\sigma _{{\text{st}}}} + {\sigma _{{\text{ct}}}}} \right)} \mathord{\left/ {\vphantom {{\left( {{\rho _{{\text{te}}}}{\sigma _{{\text{st}}}} + {\sigma _{{\text{ct}}}}} \right)} {\left( {1{\text{ + }}{\alpha _{\mathrm{E}}}{\rho _{{\text{te}}}}} \right)}}} \right. } {\left( {1{\text{ + }}{\alpha _{\mathrm{E}}}{\rho _{{\text{te}}}}} \right)}} $.

将式（11）代入式（2），并将裂缝间距取为l_m/2，可得UHPC梁钢筋在相邻裂缝间的平均应力为

${\sigma _{{\text{sm}}}} = \left( {{\sigma _{{\text{st}}}} - \kappa } \right)\frac{{\sin \left( {{{{k_1}{l_{\text{m}}}} \mathord{\left/ {\vphantom {{{k_1}{l_{\text{m}}}} 2}} \right. } 2}} \right)}}{{{{{k_1}{l_{\text{m}}}} \mathord{\left/ {\vphantom {{{k_1}{l_{\text{m}}}} 2}} \right. } 2}}} + \kappa .$

(12)

2.2 裂缝截面处钢筋应力计算

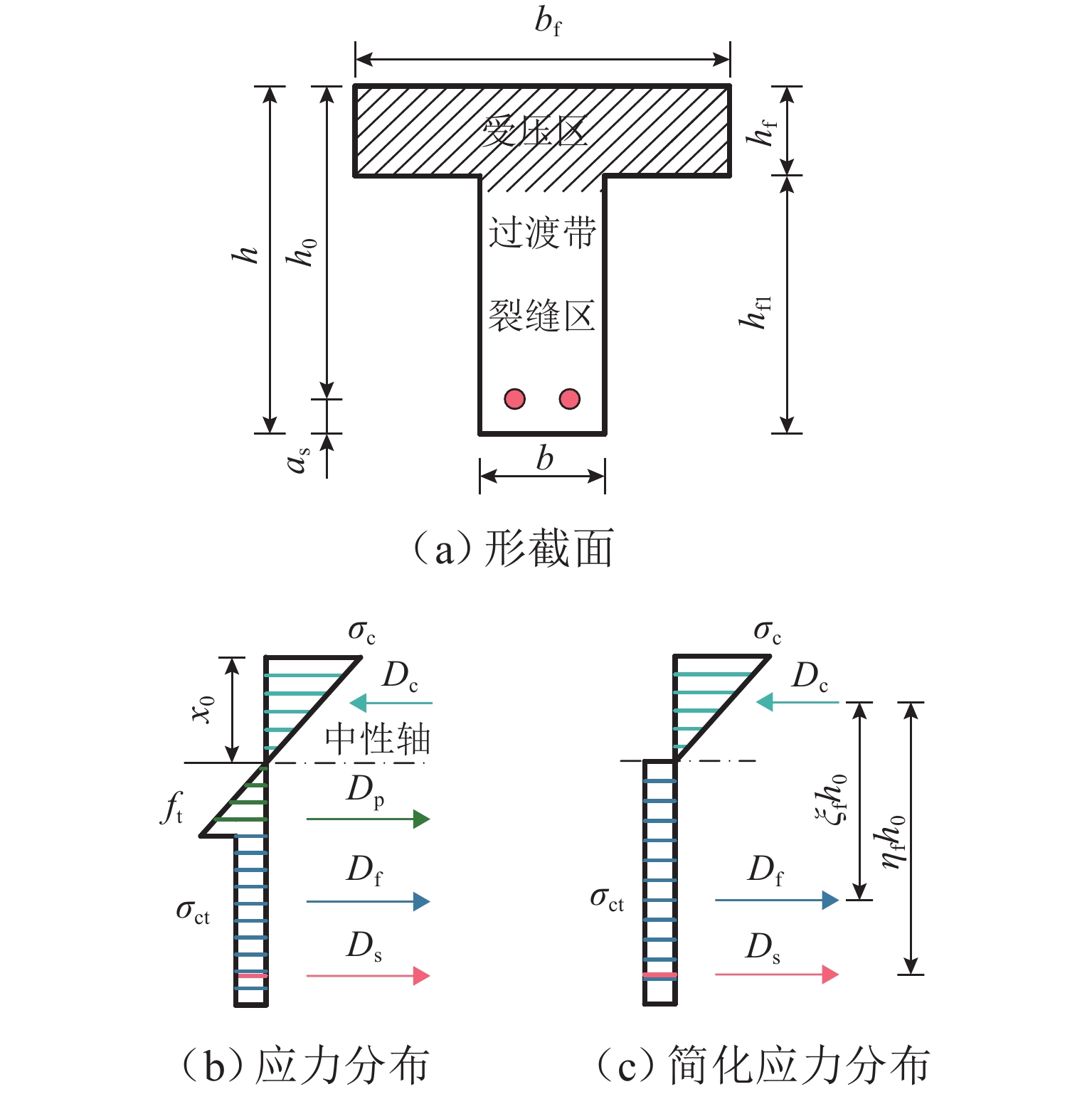
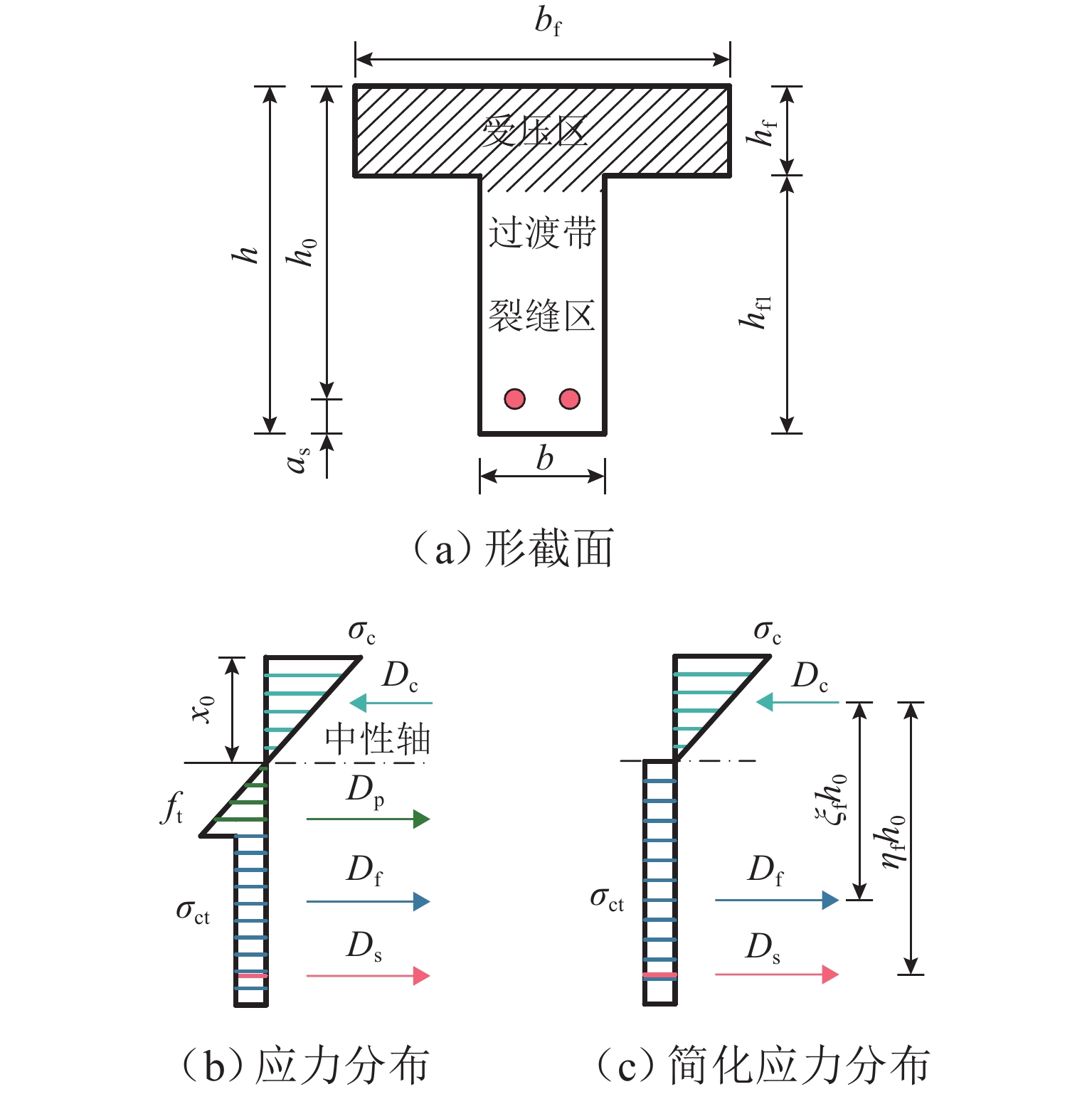
由式（12）可知，σ_st和l_m是计算σ_sm的关键参数，其中，σ_st通过裂缝截面处合力矩建立平衡关系得到. 根据裂缝截面受力的差异，将其划分为受压区、受拉区过渡带及裂缝区（图6（a）），并对各区产生的应力应变作如下基本假定：

图 6 裂缝截面的应力分布

Figure 6. Stress distribution of cracked section

下载: 全尺寸图片幻灯片

1）平均应变符合平截面假定，即沿截面高度呈线性分布；

2）受压区产生的应力主要由UHPC承担，在计算中忽略受压钢筋的作用，UHPC具有超高抗压强度和近似线弹性受压行为，其应力-应变关系在裂缝发展阶段为线弹性^[21]；

3）受拉钢筋在裂缝发展阶段未达到屈服，其应力-应变关系采用理想线弹性模型^[22]；

4） UHPC在受拉区过渡带的应力-应变关系呈线性^[23]，钢纤维在裂缝区均匀分布，且均未被拔出，因此，受拉区的应力呈上升-下降-水平分布^[24]，其中钢纤维拉应力^[24]为

${\sigma _{{\text{ct}}}} = 0.304\sqrt {{f_{{\text{cu}}}}} {\lambda _{\text{f}}} ,$

(13)

式中：$ {\lambda _{\text{f}}}{\text{ = }}{V_{\text{f}}}{{{l_{\text{f}}}} \mathord{\left/ {\vphantom {{{l_{\text{f}}}} {{d_{\text{f}}}}}} \right. } {{d_{\text{f}}}}} $，为钢纤维含量特征值，$ {{{l_{\text{f}}}} \mathord{\left/ {\vphantom {{{l_{\text{f}}}} {{d_{\text{f}}}}}} \right. } {{d_{\text{f}}}}} $为钢纤维的长径比.

根据以上基本假定，UHPC梁裂缝截面的应力分布如图6（b）、（c）所示. 图6中：D_c、D_p、D_f和D_s分别为受压区UHPC压应力、受拉区过渡带UHPC拉应力、钢纤维拉应力以及钢筋应力的合力；η_f为钢筋内力臂系数；ξ_f为钢纤维内力臂系数，用来衡量钢纤维合力至受压区合力点产生力矩的影响程度；h、h₀、h_f和h_f1分别为截面高度、截面有效高度、翼板高度和腹板高度；b_f、b分别为翼板宽度和腹板宽度；a_s为纵筋重心至腹板底板的距离.

由图6（b）可知，通过各合力矩的平衡关系即可得到σ_st，但计算公式较复杂，不便于工程化应用. 既有研究表明^[6]，采用图6（c）所示的简化应力计算得到的钢筋应力与试验值吻合良好. 因此，本文采用图6（c）所示简化应力计算裂缝截面处的钢筋应力. 将图6（c）中各合力对受压区合力点取矩，得

${\sigma _{{\text{st}}}} = \frac{{M - {D_{\text{f}}}{\xi _{\text{f}}}{h_0}}}{{{\eta _{\text{f}}}{h_0}{A_{\text{s}}}}},$

(14)

式中：D_f与UHPC梁的截面类型及尺寸有关，如式（15）.

$D_{\mathrm{f}}=\left\{\begin{array}{*{20}{l}} \left[b_{\mathrm{f}}\left(h_{\mathrm{f}}-x_0\right) + b h_{\mathrm{f1}}\right] \sigma_{\mathrm{ct}},\quad x_0 \leqslant h_{\mathrm{f}} ,\\ b\left(h-x_0\right) \sigma_{\mathrm{ct}}, \quad x_0 \gt h_{\mathrm{f}}. \end{array}\right.$

(15)

ξ_f与η_f通过梁试件裂缝截面的几何条件与内力平衡关系获得，分别如式（16）、（17）.

$\xi_{\mathrm{f}}=\left\{ \begin{array}{*{20}{l}} \dfrac{1}{2 h_0}\left[h_{\mathrm{f}}-\dfrac{x_0}{3} + \dfrac{b h_{\mathrm{f1}}\left(h-x_0\right)}{b_{\mathrm{f}}\left(h_{\mathrm{f}}-x_0\right) + b h_{\mathrm{f1}}}\right], \quad x_0 \leqslant h_{\mathrm{f}}, \\ \dfrac{1}{2 h_0}\left(h + \dfrac{x_0}{3}\right),\quad x_0 \gt h_{\mathrm{f}}, \end{array}\right.$

(16)

${\eta _{\text{f}}} = 1 - \frac{{{x_0}}}{{3{h_0}}}.$

(17)

根据式（16）、（17）可知，ξ_f、η_f的取值与x₀有关，而x₀由外荷载、配筋率及截面尺寸等因素确定，可通过图6（c）中各合力的内力平衡条件计算得到. 经计算，在 0.3倍～0.8倍极限荷载作用范围内，系数ξ_f介于0.61～0.69，平均值为0.65，变异系数为0.03；η_f介于0.84～0.90，平均值为0.87，变异系数为0.02. 由此可见，ξ_f、η_f的取值区间与离散程度均较小，基于可信度考虑，将ξ_f取为0.65，η_f取为0.87.

2.3 平均裂缝间距计算

现有裂缝间距计算理论有2种：一是无滑移理论，认为裂缝间距与c有关；另一种是黏结-滑移理论，认为裂缝间距与d_eq/ρ_te有关^[25]，其中，d_eq为纵向受拉钢筋的等效直径. 规范GB 50010—2010综合2种理论，给出普通钢筋混凝土梁的平均裂缝间距公式. 但与普通混凝土相比，UHPC中钢纤维的抗拉贡献和钢筋更优异的黏结性能能有效减小钢筋通过黏结应力传递给UHPC拉应力所需的传递长度，从而缩短裂缝间距^[26-28]. 因此，本文既考虑保护层厚度对裂缝宽度的作用，又计入黏结-滑移特性和钢纤维抗拉作用的影响. 根据修正无滑移理论中钢筋界面上裂缝宽度为0的假设，将UHPC梁的平均裂缝间距构建为

${l_{\text{m}}} = {n_1}c + \frac{{{n_2}}}{{1{\text{ + }}{n_3}{{{\lambda _{\text{f}}}\sqrt {{f_{\text{c}}}} } \mathord{\left/ {\vphantom {{{\lambda _{\text{f}}}\sqrt {{f_{\text{c}}}} } {{f_{\text{t}}}}}} \right. } {{f_{\text{t}}}}}}}\frac{{{d_{{\text{eq}}}}}}{{{\rho _{{\text{te}}}}}},$

(18)

式中：d_eq为等效钢筋直径，n₁、n₂与n₃均为待定系数.

根据本文6片UHPC梁试件的平均裂缝间距实测结果进行回归分析，可得n₁=1.75，n₂=0.08，n₃=0.10.

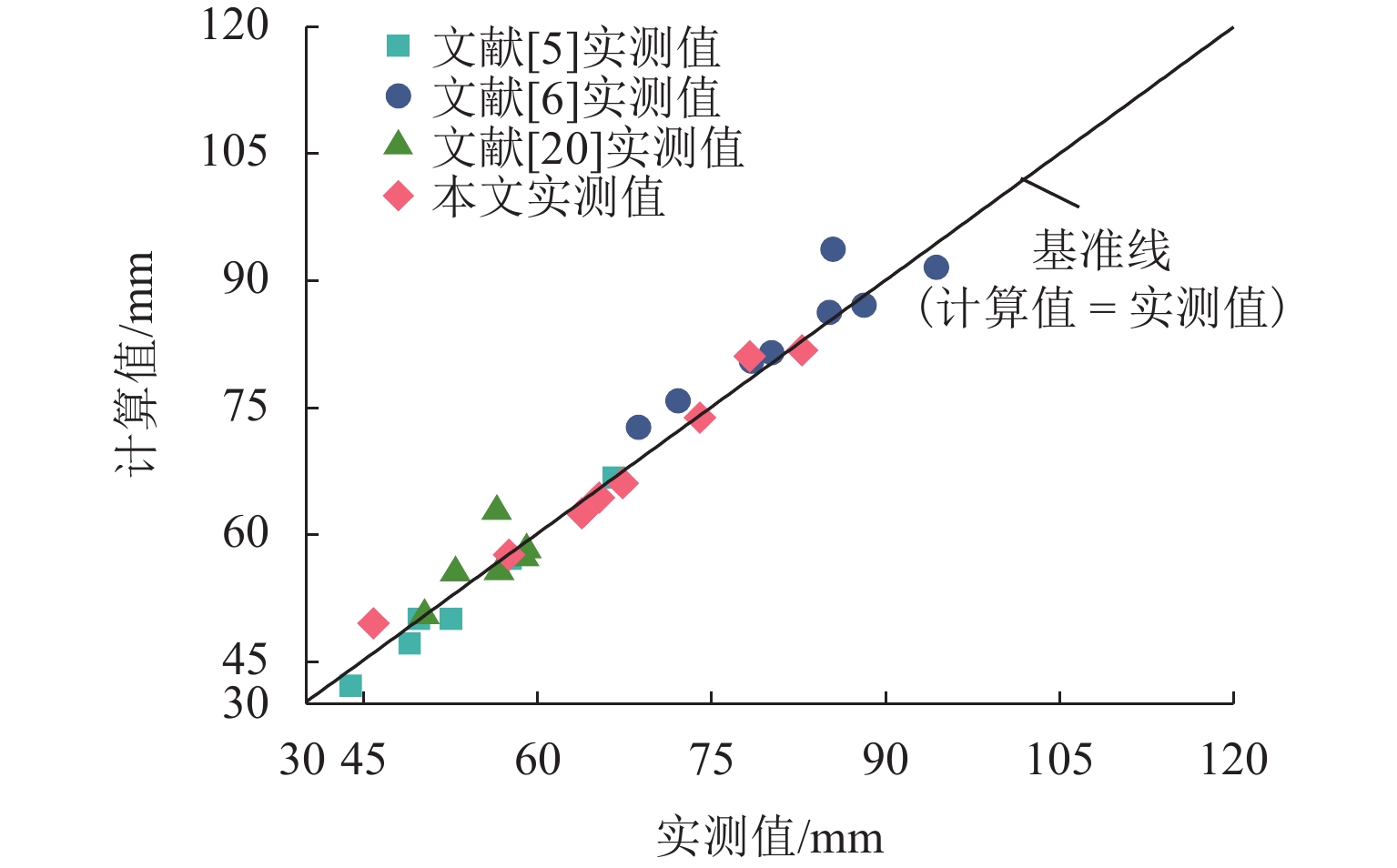
为验证平均裂缝间距计算式的正确性与适用性，将式（18）的计算值与本文、既有文献实测值进行对比，结果如图7所示.

图 7 平均裂缝间距计算值与实测值的对比

Figure 7. Comparison between calculated and measured values of average crack spacing

下载: 全尺寸图片幻灯片

由图7可知，绝大多数据点在基准线附近，表明式（18）计算值与本文、既有文献的实测值接近，其比值均值为0.99，变异系数为0.05，最大相对误差为11.17%. 由此可见，本文构建的平均裂缝间距计算式能综合考虑钢纤维体积率、配筋率、保护层厚度以及UHPC的力学性能等多种影响因素，其计算结果精度高、离散性小，适用于UHPC梁.

3. 钢筋应力计算方法的正确性验证

3.1 与本文试验结果的对比

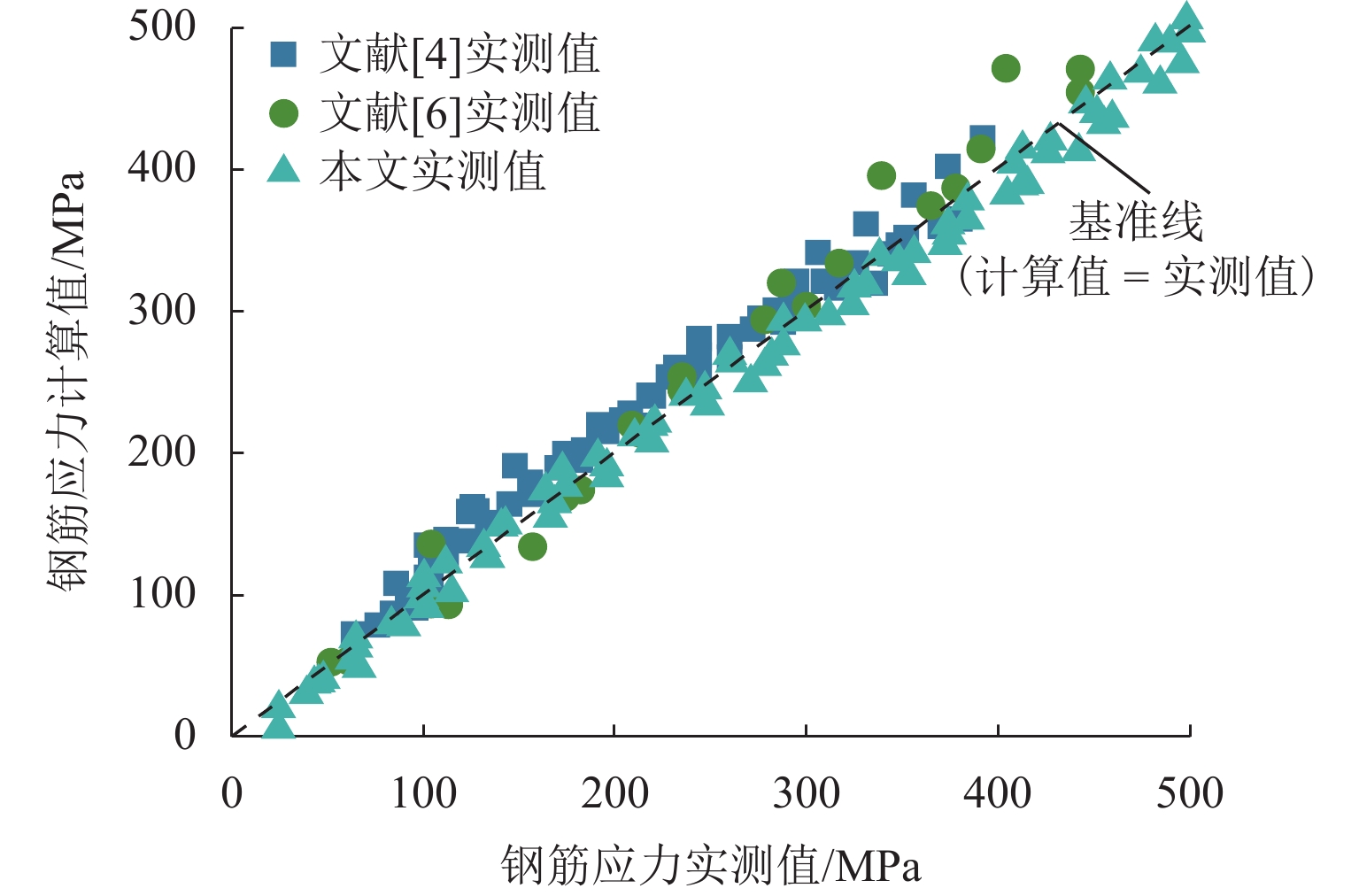
将式（14）、（18）及确定的各系数取值代入式（12），得到试件在裂缝发展阶段的钢筋平均应力，其计算值与实测值的对比结果如图8所示. 图中：L表示计算值，S表示实测值.

图 8 钢筋平均应力的对比曲线

Figure 8. Comparison curves of average reinforcement stress

下载: 全尺寸图片幻灯片

由图8可知，钢筋应力计算值与实测值之比的均值为0.99，变异系数为0.04，两者吻合良好. 随着钢纤维体积率、配筋率变化，钢筋应力与试验荷载始终呈线性关系，这是由于裂缝在发展过程中，裂缝截面钢筋应力、平均裂缝间距均与钢纤维体积率、配筋率呈线性关系.

3.2 与既有文献试验结果的对比

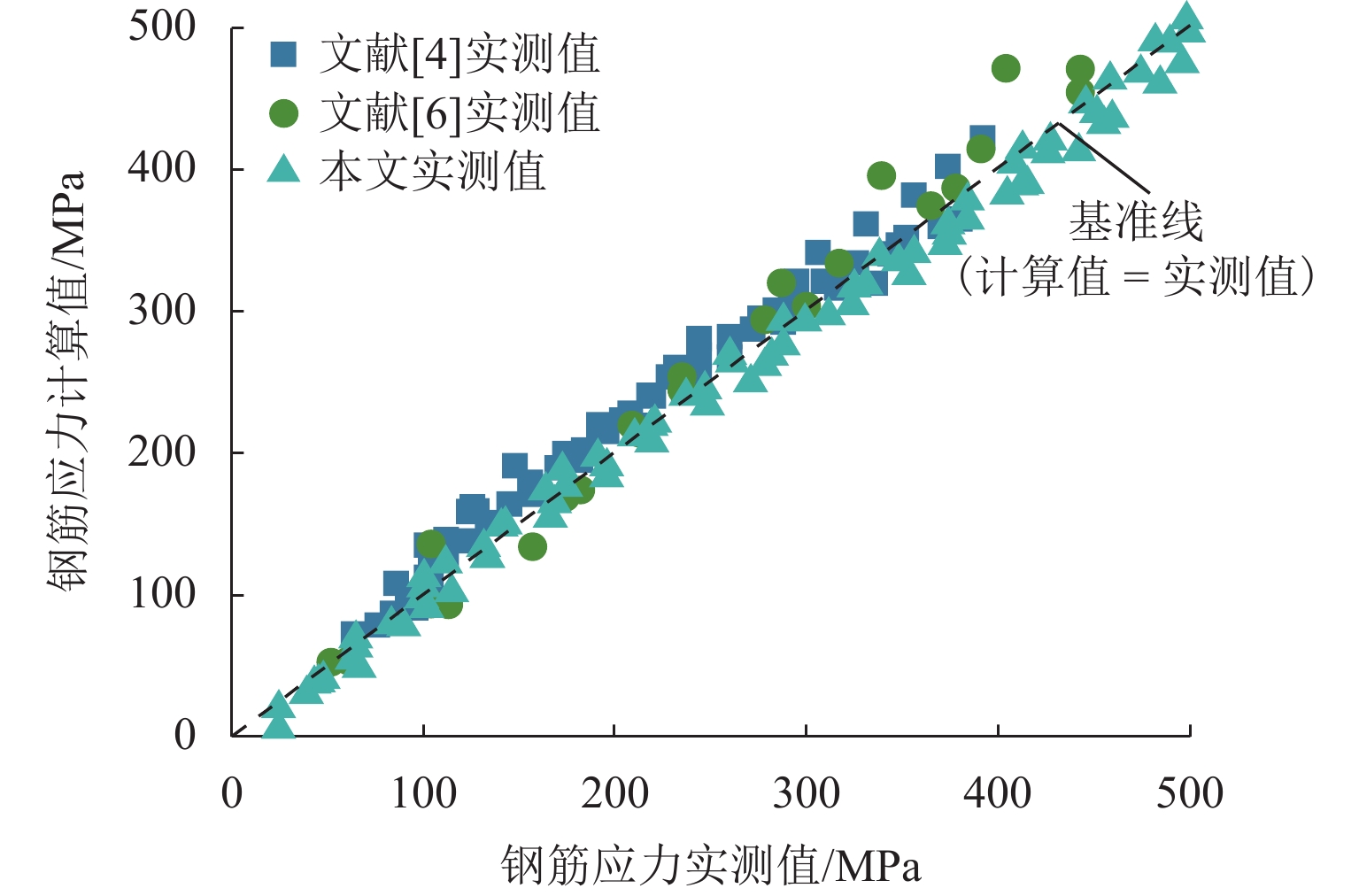
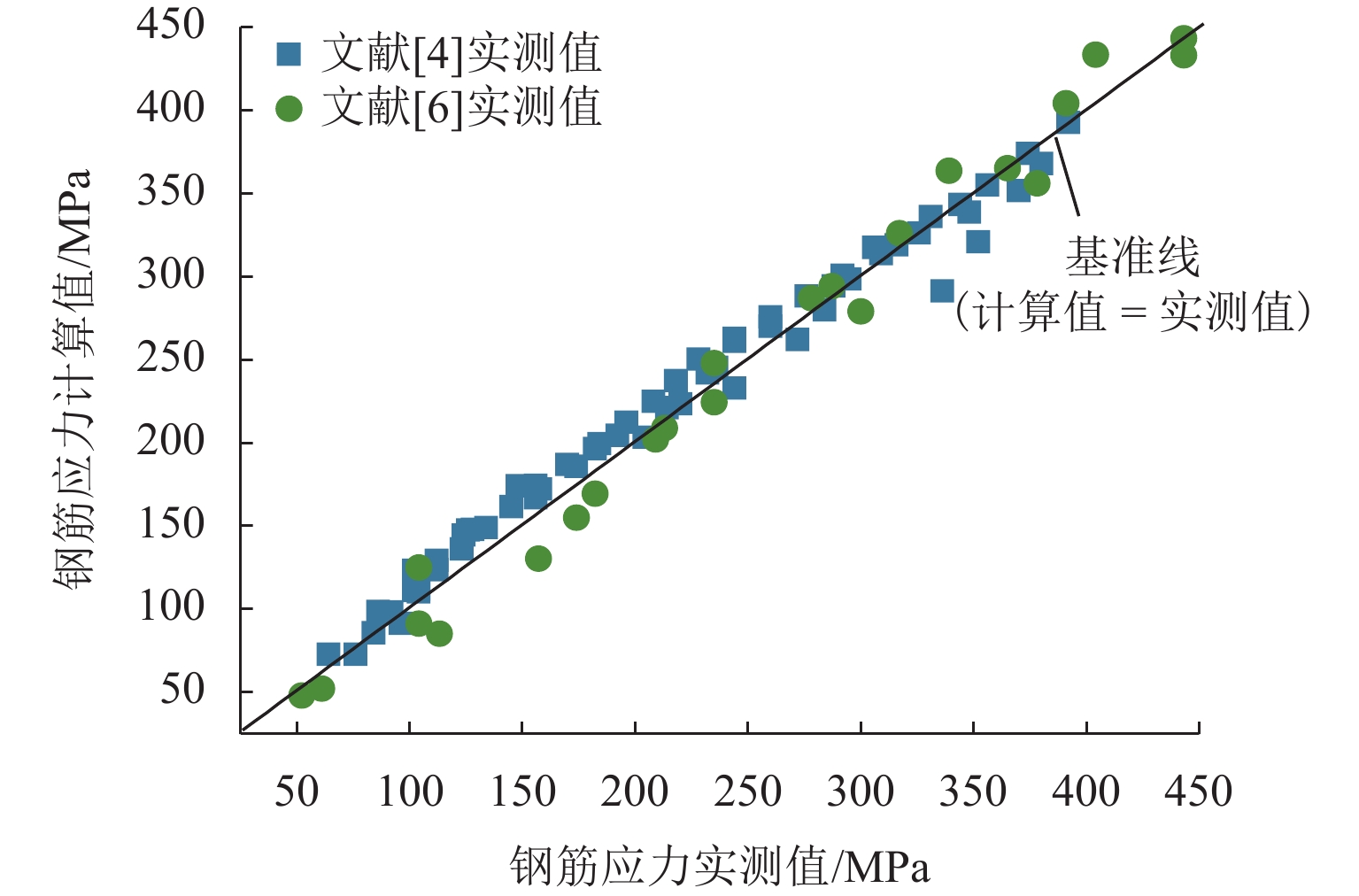
为进一步验证式（12）的适用性，收集并整理了既有文献中87组钢筋平均应力实测值，并与本文计算值进行对比，结果如图9所示.

图 9 计算值与文献实测值的对比

Figure 9. Comparison between calculated values and measured values in literature

下载: 全尺寸图片幻灯片

由图9可知，本文计算值与文献试验值之比的均值、变异系数分别为0.98、0.09. 为进一步说明计算值的准确性，考虑文献中试验值的误差影响，采用统计学中的“2sigma原则”剔除样本中的异常值，剩余样本的均值、变异系数分别为0.99、0.07. 由此可见，本文建议公式能准确计算UHPC梁的钢筋平均应力.

4. 钢筋应力计算的简化及其验证

根据上述分析可知，钢筋应力计算式（式（12））能综合考虑界面黏结-滑移性能与钢纤维抗拉贡献的影响，其计算精度较高. 但该式的计算过程繁琐，计算公式复杂，为便于实际工程应用，对式（12）作进一步简化.

4.1 应变不均匀系数简化计算

根据式（1）可知，钢筋σ_sm=$\varphi \sigma_{\mathrm{st}} $. 本文通过简化参数$\varphi $与σ_st达到简化σ_sm的目的.

根据式（12），可得

$\varphi = \left( {1 - \frac{\kappa }{{{\sigma _{{\text{st}}}}}}} \right)\frac{{\sin \left( {{{{k_1}{l_{\text{m}}}} \mathord{\left/ {\vphantom {{{k_1}{l_{\text{m}}}} 2}} \right. } 2}} \right)}}{{{{{k_1}{l_{\text{m}}}} \mathord{\left/ {\vphantom {{{k_1}{l_{\text{m}}}} 2}} \right. } 2}}} + \frac{\kappa }{{{\sigma _{{\text{st}}}}}} .$

(19)

参照规范GB 50010—2010计算模式，将$\varphi $简化为

$\varphi = {S _1}\left(1 - \frac{{0.8{M_{{\text{cr}}}}}}{{{M_{\text{s}}}}}\right) = {S _1}\left(1 - n\frac{{{f_{\text{t}}}}}{{{\rho _{{\text{te}}}}{\sigma _{{\text{st}}}}}}\right),$

(20)

式中：S₁、n均为待定系数，根据抗弯试验分析确定；M_s=η_fh₀σ_st，为钢筋应力合力矩；M_cr为抗裂弯矩，对UHPC 梁而言，计算式如式（21）.

$\begin{split} & {M_{{\text{cr}}}} = [ b(h - {x_0})(h + 2{x_0})/27 + 4bh(h - {x_0})/9 +\\ &\quad {\alpha _{{\mathrm{E}}}}{A_{\text{s}}}{\eta _{\text{f}}}{h_0} ]{f_{\text{t}}}. \end{split}$

(21)

联立式（17）与式（20），可得

${S _1} = \frac{{\left( {1 - {\kappa \mathord{\left/ {\vphantom {\kappa {{\sigma _{{\text{st}}}}}}} \right. } {{\sigma _{{\text{st}}}}}}} \right)\dfrac{{\sin \left( {{{{k_1}{l_{\text{m}}}} \mathord{\left/ {\vphantom {{{k_1}{l_{\text{m}}}} 2}} \right. } 2}} \right)}}{{{{{k_1}{l_{\text{m}}}} \mathord{\left/ {\vphantom {{{k_1}{l_{\text{m}}}} 2}} \right. } 2}}} + {\kappa \mathord{\left/ {\vphantom {\kappa {{\sigma _{{\text{st}}}}}}} \right. } {{\sigma _{{\text{st}}}}}}}}{{1 - {{0.8{M_{{\text{cr}}}}} \mathord{\left/ {\vphantom {{0.8{M_{{\text{cr}}}}} {{M_{\text{s}}}}}} \right. } {{M_{\text{s}}}}}}}.$

(22)

将式（21）、（22）与M_s的计算式代入式（20），可得

$\begin{split} & n = (({4}b(h - {x_0})(h + 2{x_0})/{27} + {{16}}bh(h - {x_0})/{9} +\\ &\quad {\alpha _{{\text{Es}}}}{A_{\text{s}}}{\eta _{\text{f}}}{h_0})) {\rho _{{\text{te}}}}/({{5{\eta _{\text{f}}}{h_0}{A_{\text{s}}}}}). \end{split}$

(23)

将试验确定的各参数值分别代入式（22）和式（23），可得系数n与S₁，计算结果如表4所示.

表 4 系数n与S₁的取值

Table 4. Values of coefficients n and S₁

梁号	n	S₁
T1	0.64	1.10
T2	0.66	0.90
T3	0.60	0.99
T4	0.62	1.17
T5	0.64	0.95
T6	0.71	0.87

下载: 导出CSV

| 显示表格

由表4可知：n的取值范围在0.60～0.71，均值为0.65，变异系数为0.03；S₁的取值范围在0.87～1.17，均值为1.00，变异系数为0.10. 由此可见，n与S₁的取值区间与离散程度均较小，基于可信度考虑，将n取为0.65，S₁取为1.00.

将n、S₁取值代入式（20），可得

$\varphi = 1 - 0.65\frac{{{f_{\text{t}}}}}{{{\rho _{{\text{te}}}}{\sigma _{{\text{st}}}}}}.$

(24)

式（24）表明，在同级别荷载作用下，UHPC梁的应变不均匀系数与普通混凝土梁相比偏小. 这是由于UHPC与钢筋有更出色的黏结性能，UHPC在未开裂截面更充分地参与工作，使得钢筋应力分布的不均匀程度更高.

4.2 裂缝截面钢筋应力简化计算

将式（15）代入式（14）可得

${\sigma _{{\text{st}}}} = \frac{M}{{0.87{A_{\text{s}}}{h_0}}} - {\beta _{\text{f}}}\frac{{{\xi _{\text{f}}}}}{{{\eta _{\text{f}}}}}\frac{{{\sigma _{{\text{ct}}}}}}{{{\rho _{{\text{te}}}}}},$

(25)

式中：β_f为与UHPC梁截面类型、尺寸有关的系数，如式（26）.

$\beta_{\mathrm{f}}=\left\{\begin{array}{*{20}{l}} \left[b_{\mathrm{f}}\left(h_{\mathrm{f}}-x_0\right) + b h_{\mathrm{f1}}\right] / b h_0, \quad x_0 \leqslant h_{\mathrm{f}}, \\ b\left(h-x_0\right) / b h_0,\quad x_0\gt h_{\mathrm{f}}. \end{array}\right.$

(26)

将各试验梁的截面参数及各级荷载作用下的受压区高度x₀代入式（26），可得β_f为0.66～0.81，平均值为0.73，变异系数为0.04. 基于可信度考虑，将β_f取为0.73.

将式（13）以及系数ξ_f、η_f、β_f的取值代入式（25）可得

${\sigma _{{\text{st}}}} = {\sigma _{\text{s}}} - \frac{{0.17{\lambda _{\text{f}}}\sqrt {{f_{{\text{cu}}}}} }}{{{\rho _{{\text{te}}}}}} \text{，}$

(27)

${\sigma _{\text{s}}} = \frac{M}{{0.87{A_{\text{s}}}{h_0}}} .$

(28)

由式（27）、（28）可知，UHPC梁的裂缝截面钢筋应力计算式与普通混凝土梁相协调，即当钢纤维体积率为0时，两者的计算公式一致；随着钢纤维体积率提升，钢纤维分担的应力增加，UHPC梁的裂缝截面钢筋应力与普通混凝土梁相比减小.

4.3 简化公式的正确性验证

根据上述分析可知，式（24）与式（27）的乘积即为钢筋平均应力的简化公式，即

${\sigma _{{\text{sm}}}} = {\sigma _{{\text{st}}}} - 0.65{{{f_{\text{t}}}}}/{{{\rho _{{\text{te}}}}}}.$

(29)

由式（26）计算得到的钢筋平均应力与本文、既有文献实测值的对比结果如图10所示. 由图10可知，简化公式计算值与实测值之比的均值为1.03，变异系数为0.06，两者吻合良好. 因此，本文建议的简化公式计算精度较高，适用于UHPC梁的钢筋平均应力计算.

图 10 简化计算值与文献实测值的对比

Figure 10. Comparison between calculated values of simplified formula and measured values in literature

下载: 全尺寸图片幻灯片

5. 结　论

本文通过微元体建立平衡、变形与黏结-滑移微分方程，导出相邻裂缝间钢筋平均应力的计算式，并对其进行简化，得到如下主要结论：

1）随着钢纤维体积率的提高，钢筋应力减小，但不改变单位荷载下钢筋应力的增幅；随着配筋率的提高，钢筋应力及单位荷载下钢筋应力的增幅均减小.

2）与普通混凝土梁相比，UHPC梁的钢筋应变不均匀系数偏小，说明UHPC梁钢筋应力在相邻裂缝间分布的不均匀程度更高；掺入的钢纤维在裂缝截面分担了部分应力，使裂缝截面处的钢筋应力减小.

3）建立的钢筋应力计算式能充分考虑钢筋与UHPC界面黏结-滑移特性及钢纤维抗拉贡献的影响，其计算值与本文、既有文献实测值吻合良好.

4）为便于工程化应用，通过简化钢筋应变不均匀系数及裂缝截面钢筋应力，进一步提出钢筋应力简化公式，其计算值与实测值吻合良好.

图 1 梁的配筋及截面尺寸

Figure 1. Reinforcement and section dimensions of specimens

下载: 全尺寸图片幻灯片

图 2 四点抗弯试验

Figure 2. Four-point bending test

下载: 全尺寸图片幻灯片

图 3 荷载-钢筋应力关系曲线

Figure 3. Load-reinforcement stress relationship curves

下载: 全尺寸图片幻灯片

图 4 受弯构件开裂后的变形及受力

Figure 4. Deformation and stress of bending member after cracking

下载: 全尺寸图片幻灯片

图 5 隔离体及微段的应力分布

Figure 5. Stress distribution of isolator and micro-segment

下载: 全尺寸图片幻灯片

图 6 裂缝截面的应力分布

Figure 6. Stress distribution of cracked section

下载: 全尺寸图片幻灯片

图 7 平均裂缝间距计算值与实测值的对比

Figure 7. Comparison between calculated and measured values of average crack spacing

下载: 全尺寸图片幻灯片

图 8 钢筋平均应力的对比曲线

Figure 8. Comparison curves of average reinforcement stress

下载: 全尺寸图片幻灯片

图 9 计算值与文献实测值的对比

Figure 9. Comparison between calculated values and measured values in literature

下载: 全尺寸图片幻灯片

图 10 简化计算值与文献实测值的对比

Figure 10. Comparison between calculated values of simplified formula and measured values in literature

下载: 全尺寸图片幻灯片

表 1 试件的编号与参数

Table 1. Number and parameters of specimens

变量	梁号	V_f/%	纵筋配置	ρ_s/%	c/mm
标准梁	T1	2	216	1.60	15
钢纤维体积率	T2	1	216	1.60
钢纤维体积率	T3	3	216	1.60
配筋率	T4	2	212	0.89
	T5		220	2.51
	T6		416	3.20

下载: 导出CSV

表 2 基本力学指标

Table 2. Basic mechanical indicators

V_f/%	f_cu/MPa	f_c/MPa	f_t/MPa	E_c/GPa
1	121.22	83.72	6.35	41
2	133.71	89.14	7.84	42
3	141.53	97.82	9.32	44

下载: 导出CSV

表 3 钢筋应力的实测结果

Table 3. Measured results of reinforcement stress

梁号	F_cr/kN	F_t/kN	σ_cr/MPa	σ_t/MPa	V_m/（MPa•kN⁻¹）
T1	55.24	321.42	37.77	593.75	2.09
T2	37.27	311.31	32.23	602.22	2.08
T3	69.72	334.27	44.53	579.63	2.02
T4	43.43	218.51	41.82	581.70	3.08
T5	60.67	454.88	48.34	579.06	1.35
T6	61.14	529.20	39.25	578.51	1.15

下载: 导出CSV

表 4 系数n与S₁的取值

Table 4. Values of coefficients n and S₁

梁号	n	S₁
T1	0.64	1.10
T2	0.66	0.90
T3	0.60	0.99
T4	0.62	1.17
T5	0.64	0.95
T6	0.71	0.87

下载: 导出CSV

参考文献(28)

[1]	SHIRAI K, YIN H, TEO W. Flexural capacity prediction of composite RC members strengthened with UHPC based on existing design models[J]. Structures, 2020, 23: 44-55. doi: 10.1016/j.istruc.2019.09.017
[2]	ELSAYED M, BADAWY S, TAYEH B A, et al. Shear behaviour of ultra-high performance concrete beams with openings[J]. Structures, 2022, 43(6): 546-558.
[3]	WANG J Q, QI J N, TONG T, et al. Static behavior of large stud shear connectors in steel-UHPC composite structures[J]. Engineering Structures, 2019, 178: 534-542. doi: 10.1016/j.engstruct.2018.07.058
[4]	中华人民共和国住房和城乡建设部. 混凝土结构设计规范:GB 50010—2010[S]. 北京:中国建筑工业出版社,2010.
[5]	邱明红,邵旭东,胡伟业,等. 钢筋UHPC受弯构件裂缝宽度计算方法研究[J]. 土木工程学报,2020,53(10): 89-98,119. QIU Minghong, SHAO Xudong, HU Weiye, et al. Calculation method for crack width of reinforced UHPC flexural components[J]. China Civil Engineering Journal, 2020, 53(10): 89-98,119.
[6]	邱明红,邵旭东,胡伟业,等. 钢筋UHPC矩形截面受弯构件的钢筋应力简化计算[J]. 中国公路学报,2021,34(8): 106-117. QIU Minghong, SHAO Xudong, HU Weiye, et al. Simplified calculation of reinforcement stress in reinforced UHPC bending members with rectangular sections[J]. China Journal of Highway and Transport, 2021, 34(8): 106-117.
[7]	郑文忠,李莉,卢姗姗. 钢筋活性粉末混凝土简支梁正截面受力性能试验研究[J]. 建筑结构学报,2011,32(6): 125-134. ZHENG Wenzhong, LI Li, LU Shanshan. Experimental research on mechanical performance of normal section of reinforced reactive powder concrete beam[J]. Journal of Building Structures, 2011, 32(6): 125-134.
[8]	赵国藩,王清湘. 钢筋混凝土构件裂缝宽度分析的应力图形和计算模式[J]. 大连工学院学报,1984,24(4): 87-94. ZHAO Guofan, WANG Qingxiang. Stress distribution and calculation formula for crack width analysis of reinforced concrete members[J]. Journal of Dalian University of Technology, 1984, 24(4): 87-94.
[9]	朱虹,董志强,吴刚,等. FRP筋混凝土梁的刚度试验研究和理论计算[J]. 土木工程学报,2015,48(11): 44-53. ZHU Hong, DONG Zhiqiang, WU Gang, et al. Experimental study and theoretical calculation on the flexural stiffness of concrete beams reinforced with FRP bars[J]. China Civil Engineering Journal, 2015, 48(11): 44-53.
[10]	贾方方. 钢筋与活性粉末混凝土粘结性能的试验研究[D]. 北京:北京交通大学,2013.
[11]	徐海宾,邓宗才. UHPC梁开裂弯矩和裂缝试验[J]. 哈尔滨工业大学学报,2014,46(4): 87-92. XU Haibin, DENG Zongcai. Cracking moment and crack width of ultra-high performance concrete beams[J]. Journal of Harbin Institute of Technology, 2014, 46(4): 87-92.
[12]	YOO D Y, YOON Y S. A review on structural behavior, design, and application of ultra-high-performance fier-reinforced concrete[J]. International Journal of Concrete Structures and Materials, 2016, 10(2): 125-142. doi: 10.1007/s40069-016-0143-x
[13]	HASGUL U, TURKER K, BIROL T, et al. Flexural behavior of ultra-high-performance fiber reinforced concrete beams with low and high reinforcement ratios[J]. Structural Concrete, 2018, 19(6): 1577-1590. doi: 10.1002/suco.201700089
[14]	AHMED K S, HABIB M A, ASEF M F. Flexural response of stainless steel reinforced concrete beam[J]. Structures, 2021, 34: 589-603. doi: 10.1016/j.istruc.2021.08.019
[15]	中华人民共和国住房和城乡建设部. 活性粉末混凝土:GB/T 31387—2015 [S]. 北京:中国标准出版社,2015.
[16]	中国建筑科学研究院. 混凝土结构试验方法标准:GB/T 50152—2012[S]. 北京:中国建筑工业出版社,2012.
[17]	高丹盈,张明,赵军. 疲劳荷载下钢纤维高强混凝土梁裂缝宽度的计算方法[J]. 土木工程学报,2013,46(3): 40-48. GAO Danying, ZHANG Ming, ZHAO Jun. Calculating method for crack width of steel fiber reinforced high-strength concrete beams under fatigue loads[J]. China Civil Engineering Journal, 2013, 46(3): 40-48.
[18]	宁喜亮,丁一宁. 钢筋钢纤维自密实混凝土梁裂缝宽度试验研究[J]. 工程力学,2017,34(4): 116-124. NING Xiliang, DING Yining. Experimental research on crack width of steel fibers reinforced self-consolidating concrete beams[J]. Engineering Mechanics, 2017, 34(4): 116-124.
[19]	高丹盈,刘建秀. 钢纤维混凝土基本理论[M]. 北京:科学技术文献出版社,1994.
[20]	贾方方,安明喆,余自若,等. 钢筋与活性粉末混凝土黏结性能的梁式试验研究[J]. 铁道学报,2012,34(6): 83-87. JIA Fangfang, AN Mingzhe, YU Ziruo, et al. Beam test of bond behavior between steel bars and reactive powder concrete[J]. Journal of the China Railway Society, 2012, 34(6): 83-87.
[21]	BABY F, MARCHAND P, ATRACH M, et al. Analysis of flexure-shear behavior of UHPFRC beams based on stress field approach[J]. Engineering Structures, 2013, 56: 194-206. doi: 10.1016/j.engstruct.2013.04.024
[22]	徐明雪,梁兴文,汪萍,等. 超高性能混凝土梁正截面受弯承载力理论研究[J]. 工程力学,2019,36(8): 70-78. XU Mingxue, LIANG Xingwen, WANG Ping, et al. Theoretical investigation on normal section flexural capacity of uhpc beams[J]. Engineering Mechanics, 2019, 36(8): 70-78.
[23]	BAE B I, CHOI H K, CHOI C S. Flexural strength evaluation of reinforced concrete members with ultra high performance concrete[J]. Advances in Materials Science and Engineering, 2016, 2016: 2815247.1-2815247.11.
[24]	王景全,戚家南,刘加平. 基于细观本构模型的UHPC梁受弯全过程分析[J]. 建筑结构学报,2020,41(9): 137-144. WANG Jingquan, QI Jianan, LIU Jiaping. Flexural analysis of UHPC beams based on a mesoscale constitutive model[J]. Journal of Building Structures, 2020, 41(9): 137-144.
[25]	周建民,王眺,赵勇,等. 高强钢筋混凝土受弯构件裂缝宽度计算方法的研究[J]. 土木工程学报,2010,43(9): 69-76. ZHOU Jianmin, WANG Tiao, ZHAO Yong, et al. Research on the calculation of crack width for RC flexural member using high-strength bars[J]. China Civil Engineering Journal, 2010, 43(9): 69-76.
[26]	程东辉,范永萱,王彦松. RC类活性粉末混凝土钢筋粘结-滑移本构模型[J]. 吉林大学学报(工学版),2021,51(4): 1317-1330. CHENG Donghui, FAN Yongxuan, WANG Yansong. Bond-slip constitutive model of steel bars and reactive powder concrete under standard curing[J]. Journal of Jilin University (Engineering and Technology Edition), 2021, 51(4): 1317-1330.
[27]	张哲,李帅帅,朱平,等. 钢筋-超高性能混凝土界面黏结性能试验[J]. 湖南大学学报(自然科学版),2022,49(11): 105-115. ZHANG Zhe, LI Shuaishuai, ZHU Ping, et al. Test on interface bonding behavior between steel rebar and ultra-high performance concrete[J]. Journal of Hunan University (Natural Sciences), 2022, 49(11): 105-115.
[28]	梁芮,黄远. 高强钢筋与超高性能混凝土黏结性能试验研究[J]. 建筑结构学报,2022,43(9): 294-302. LIANG Rui, HUANG Yuan. Experimental study on bond performance between high strength steel rebar and ultra-high performance concrete[J]. Journal of Building Structures, 2022, 43(9): 294-302.

施引文献

附加材料(0)

访问统计

点击查看大图

图(10) / 表(4)

计量

文章访问数: 275
HTML全文浏览量: 113
PDF下载量: 50
被引次数: 0

1. 试验研究
1.1 试验设计及加载方案
1.2 钢筋应力的发展过程及变化规律分析
2. UHPC梁的钢筋应力计算
2.1 相邻裂缝间钢筋应力计算
2.2 裂缝截面处钢筋应力计算
2.3 平均裂缝间距计算
3. 钢筋应力计算方法的正确性验证
3.1 与本文试验结果的对比
3.2 与既有文献试验结果的对比
4. 钢筋应力计算的简化及其验证
4.1 应变不均匀系数简化计算
4.2 裂缝截面钢筋应力简化计算
4.3 简化公式的正确性验证
5. 结　论

1. 试验研究
1.1 试验设计及加载方案
1.2 钢筋应力的发展过程及变化规律分析
2. UHPC梁的钢筋应力计算
2.1 相邻裂缝间钢筋应力计算
2.2 裂缝截面处钢筋应力计算
2.3 平均裂缝间距计算
3. 钢筋应力计算方法的正确性验证
3.1 与本文试验结果的对比
3.2 与既有文献试验结果的对比
4. 钢筋应力计算的简化及其验证
4.1 应变不均匀系数简化计算
4.2 裂缝截面钢筋应力简化计算
4.3 简化公式的正确性验证
5. 结　论

参考文献(28)

施引文献

附加材料(0)

访问统计

考虑黏结-滑移效应的UHPC梁钢筋应力计算方法

doi: 10.3969/j.issn.0258-2724.20230130

作者简介: 孙永新（1989—），男，讲师，博士研究生，研究方向为UHPC桥梁结构设计理论与工程应用，E-mail：syx170007@163.com

通讯作者: 蔺鹏臻（1977—），男，教授，博士生导师，研究方向为大跨度桥梁设计理论及建造技术，E-mail：pzhlin@mail.lzjtu.cn

计量

出版历程

Calculation Method for Reinforcement Stress in Ultra-High Performance Concrete Beams Considering Bond-Slip Effect

1. 试验研究

1.1 试验设计及加载方案

1.2 钢筋应力的发展过程及变化规律分析

1.2.1 钢筋应力的发展过程

1.2.2 钢筋应力变化规律分析

2. UHPC梁的钢筋应力计算

2.1 相邻裂缝间钢筋应力计算

2.2 裂缝截面处钢筋应力计算

2.3 平均裂缝间距计算

3. 钢筋应力计算方法的正确性验证

3.1 与本文试验结果的对比

3.2 与既有文献试验结果的对比

4. 钢筋应力计算的简化及其验证

4.1 应变不均匀系数简化计算

4.2 裂缝截面钢筋应力简化计算

4.3 简化公式的正确性验证

5. 结 论

计量

出版历程

目录

1. 试验研究

1.1 试验设计及加载方案

1.2 钢筋应力的发展过程及变化规律分析

2. UHPC梁的钢筋应力计算

2.1 相邻裂缝间钢筋应力计算

2.2 裂缝截面处钢筋应力计算

2.3 平均裂缝间距计算

3. 钢筋应力计算方法的正确性验证

3.1 与本文试验结果的对比

3.2 与既有文献试验结果的对比

4. 钢筋应力计算的简化及其验证

4.1 应变不均匀系数简化计算

4.2 裂缝截面钢筋应力简化计算

4.3 简化公式的正确性验证

5. 结 论

作者简介:
孙永新（1989—），男，讲师，博士研究生，研究方向为UHPC桥梁结构设计理论与工程应用，E-mail：syx170007@163.com

通讯作者:
蔺鹏臻（1977—），男，教授，博士生导师，研究方向为大跨度桥梁设计理论及建造技术，E-mail：pzhlin@mail.lzjtu.cn

5. 结　论

5. 结　论