Vibration Reduction of Bearing-Rotor with Electromagnetic Damper Considering Dynamic Stiffness
-
摘要:
为了有效抑制转子系统的不平衡振动,本文提出将考虑动态刚度的电磁阻尼器应用于转子系统中. 首先,建立转子系统运动方程并对其进行无量纲化;其次,基于等效磁路法,建立了考虑涡流效应的电磁阻尼器模型,分析了涡流效应对电磁阻尼器刚度的影响,并采用非线性动态自适应惯性权重的PSO算法对电磁阻尼器的PID (比例、积分、微分)3个控制参数进行优化;最后,对转子的动力学特性进行了分析. 研究结果表明:在涡流效应的影响下,电磁阻尼器的刚度是动态变化的,当转速为100 kHz时,理论上位移刚度和电流刚度分别下降了10.0%、6.6%;通过非线性动态自适应惯性权重的PSO算法优化得到的PID参数响应快,超调量小,能够在0.1 s内迅速地将圆盘的偏离量调至0,具有良好的控制效果;相比于静态刚度,考虑动态刚度后,圆盘的振幅有所增大,当转速为4782 Hz时,圆盘的振幅增大了5.33%;偏心距增大会导致圆盘的振幅增大,当转速超过242 Hz时,圆盘振幅增大的幅度与圆盘偏心距增大的幅度几乎成正比.
Abstract:In order to suppress the unbalance vibration of the rotor system effectively, the electromagnetic damper considering dynamic stiffness was applied to the rotor system in this paper. Firstly, the motion equations of the rotor system were established and nondimensionalized; secondly, based on the equivalent magnetic circuit method, the electromagnetic damper model considering the eddy-current effect was established, and the influence of the eddy-current effect on the stiffness of the electromagnetic damper was analyzed. The particle swarm optimization (PSO) algorithm with nonlinear dynamic adaptive inertia weight was used to optimize the three control parameters of the proportion integration differentiation (PID) of the electromagnetic damper; finally, the dynamic characteristics of the rotor were analyzed. The results indicate that under the influence of the eddy-current effect, the stiffness of the electromagnetic damper dynamically changes. When the speed is 100 kHz, the displacement stiffness and current stiffness decrease by 10.0% and 6.6% respectively; the PID parameters optimized by the PSO algorithm with nonlinear dynamic adaptive inertia weight have great control effects, such as fast response and small overshoot, and they can quickly adjust the deviation of the disk to 0 within 0.1 seconds; compared with static stiffness, the amplitude of the disk increases slightly after considering dynamic stiffness. When the speed is 4 782 Hz, the amplitude of the disk increases by 5.33%; the increase in eccentricity will lead to an increase in the amplitude of the disk. When the speed exceeds 242 Hz, the amplitude of the disk increases almost proportionally to that of the disk eccentricity.
-
Key words:
- electromagnetic damper /
- magnetic circuit /
- eddy-current /
- dynamic stiffness
-
随着工业的不断发展,高速电机对转子系统的性能需求越来越高,使得转子系统朝着高转速、高精度、高稳定性的方向发展[1]. 然而转子在加工制造过程中难免出现误差,同时转子在运行过程中也会发生磨损,会导致转子质量偏心,转子系统在运行过程中因为偏心力的作用而剧烈振动[2-5]. 因此抑制转子振动,确保其平稳运行至关重要.
相比于传统的黏滞、黏弹性阻尼器,电磁阻尼器具有无摩擦、无须润滑、噪声小、低污染等优点[6-7],在工程领域中的应用非常广泛,如车辆减振器、航天器对接的减振、机器人减振系统、高速列车的制动等[7-9]. 文献[10]研究了电磁阻尼器对输液管道性能的影响,结果表明电磁阻尼器能降低管道的振动;文献[11]分析了带电磁阻尼器的裂纹转子系统的动力学特性,结果表明该动力学特性比传统裂纹转子系统的动力学特性更为复杂;文献[12]设计了一种用于控制高速旋转轴横向转子振动的主动电磁阻尼器,并与挤压薄膜阻尼器对比,结果表明主动电磁阻尼器比挤压薄膜阻尼器更有效地降低了横向转子振动;文献[13]提出一种用于调节永磁同步电机转子振动响应的电磁减振器,实验结果证实电磁减振器可用于抑制带负载转子的高速电机的振动响应,并且基于位移反馈的电磁减振器拓宽了减振的有效范围. 在上述研究中,电磁阻尼器的刚度(位移刚度和动态刚度)都被视为常数. 然而,电磁阻尼器在高速运转时会在定子、转子中产生涡流,这不仅会产生损耗带来热问题,而且影响动态电磁力和动态刚度系数,从而影响到系统的动态特性[14-15]. 因此,为了有效减小系统误差,考虑动态刚度的影响是很有必要的.
基于以上研究,本文提出考虑涡流效应的电磁阻尼器,根据等效磁路法推导电磁阻尼器的动态刚度,采用非线性动态自适应惯性权重的粒子群优化算法(PSO)对电磁阻尼器的比例、积分、微分(PID)控制参数进行优化,建立带主动电磁阻尼器(AMD)的转子系统的动力学模型,并在Simulink中进行仿真,分析了涡流效应下转子系统的动力学特性.
1. 带主动电磁阻尼器的转子系统模型
1.1 转子系统模型
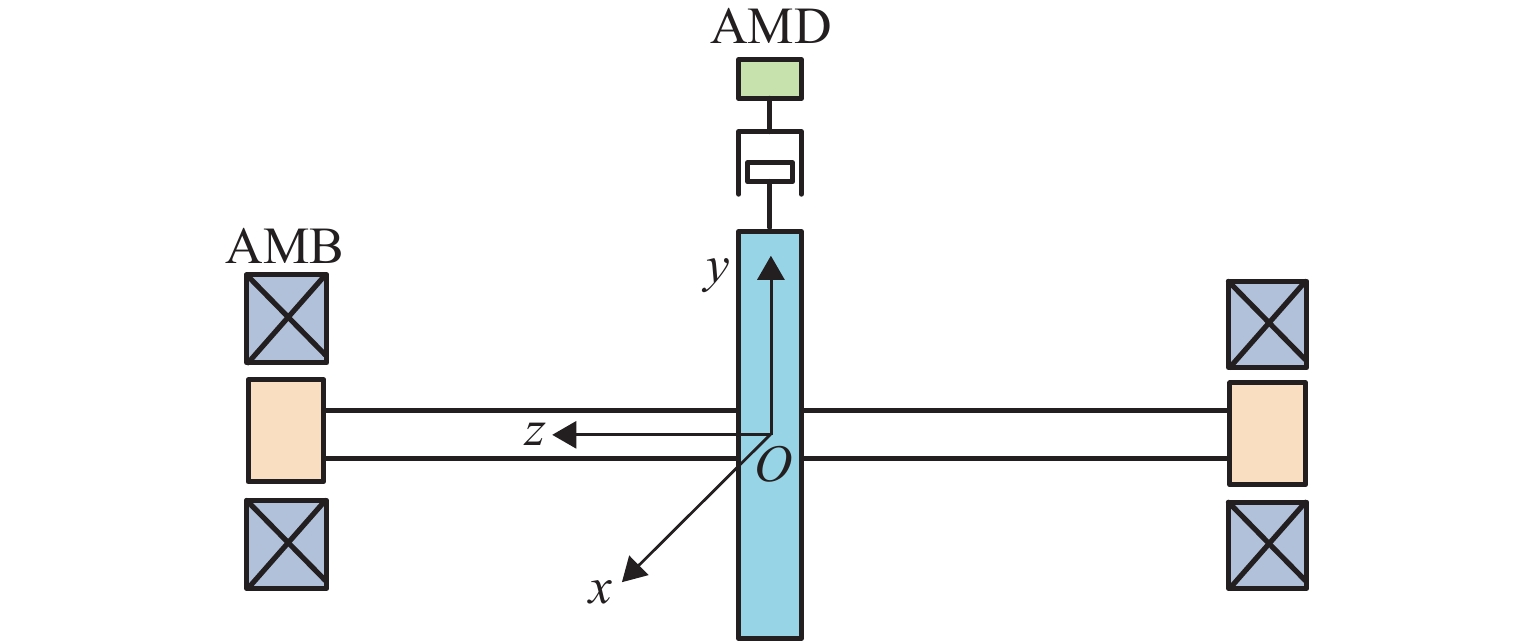
本文研究的转子系统由轴颈、转轴和圆盘组成,如图1所示. 转轴两端采用主动电磁轴承(AMB)支撑,圆盘位于转轴的中间, AMD安装在圆盘位置,对圆盘的振动进行控制. 关于转子的假设如下:
1) 转轴只在xOy平面内运动,忽略轴向位移;
2) 转轴是柔性的,圆盘是刚性的;
3) 转轴的扭转刚度很大,即不计扭转振动.
1.2 转子系统的运动方程
稳态运行条件下,转子系统在固定直角坐标系xOy中的运动方程为
{m1¨x1+2k(x1−x2)=fx+F1x,m1¨y1+2k(y1−y2)=fy−m1g+F1y,m2¨x2+k(x2−x1)=F2x,m2¨y2+k(y2−y1)=−m2g+F2y, (1) 式中:x1、y1(x2、y2)分别为圆盘(轴颈)x、y方向的位移;m1、m2分别为圆盘、轴颈的质量;fx、fy分别为圆盘的偏心质量在x、y方向的偏心力,如式(2);k为转子的刚度;F1x、F1y分别为电磁阻尼器在x、y方向的电磁力;F2x、F2y分别为电磁轴承在x、y方向的电磁力,电磁轴承提供的电磁力求解方法同电磁阻尼器.
[fxfy]=m1eω2[cos(ωt+φ)sin(ωt+φ)], (2) 式中:e为圆盘的偏心距;ω为转轴的角速度;φ为初始相位角,t为时间.
采用PID方法对转子进行控制,控制电流与位移的关系为
{i1x=−(kP1x1+kI1∫x1dt+kD1˙x1),i1y=−(kP1y1+kI1∫y1dt+kD1˙y1),i2x=−(kP2x2+kI2∫x2dt+kD2˙x2),i2y=−(kP2y2+kI2∫y2dt+kD2˙y2), (3) 式中:i1x、i1y(i2x、i2y)分别为电磁阻尼器(电磁轴承)x、y方向的控制电流;kP1、kI1和kD1(kP2、kI2和kD2)分别为电磁阻尼器(电磁轴承)控制器的比例增益、积分增益和微分增益因子.
1.3 转子系统的无量纲运动方程
为便于分析,将运动方程进行无量纲化处理,即X1=x1/C1,Y1=y1/C1,X2=x2/C2,Y2=y2/C2,τ=ωnt,ωn=√k/M,其中:C1为电磁阻尼器的气隙长度;C2为电磁轴承的气隙长度;M=m1+2m2为转子系统的总质量. 将式(1)的前两式同除以Mω2nC1,后两式同除以Mω2nC2,并令U=e/C1,转速比Ω=ω/ωn,α1=m1/M,α2=m2/M,β=C1/C2,γ1=g/(ω2nC1),γ2=g/(ω2nC2),¯k1=K1/k,¯k2=K2/k,¯G1=G1/(kC1),¯G2=G2/(kC2),其中,K1和G1(K2和G2)分别为电磁阻尼器(电磁轴承)的位移刚度和电流刚度.
将式(1)进行无量纲化后得:
{¨X1+2(X1−1βX2)/α1=Ω2Ucos(Ωτ)−¯k1α1X1+¯G1α1i1x,¨Y1+2(Y1−1βY2)/α1=Ω2Usin(Ωτ)−γ1−¯k1α1Y1+¯G1α1i1y,¨X2+(X2−βX1)α2=−¯k2α2X2+¯G2α2i2x,¨Y2+(Y2−βY1)α2=−γ2−¯k2α2Y2+¯G2α2i2y. (4) 2. 涡流效应下电磁阻尼器的动态特性
2.1 电磁阻尼器模型
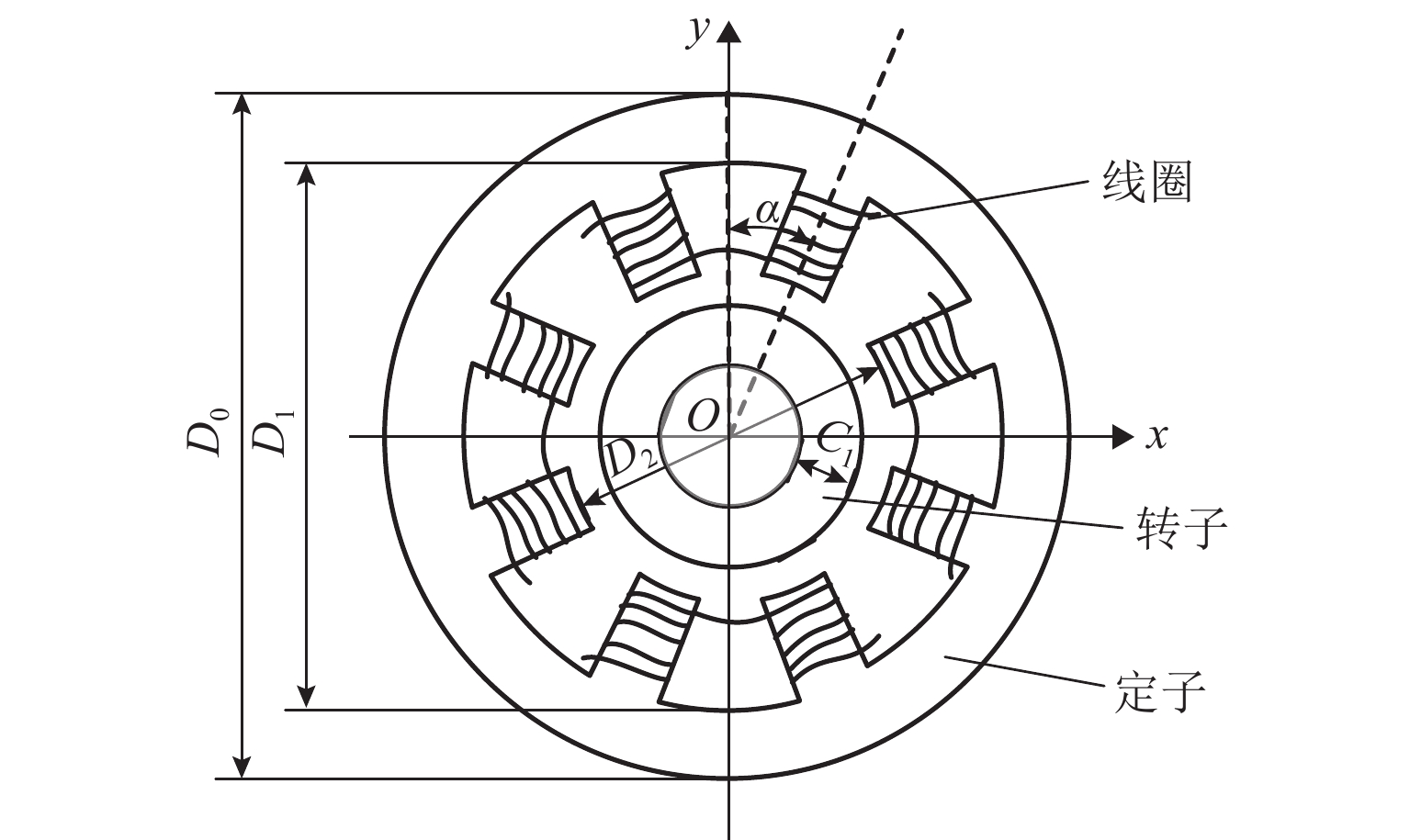
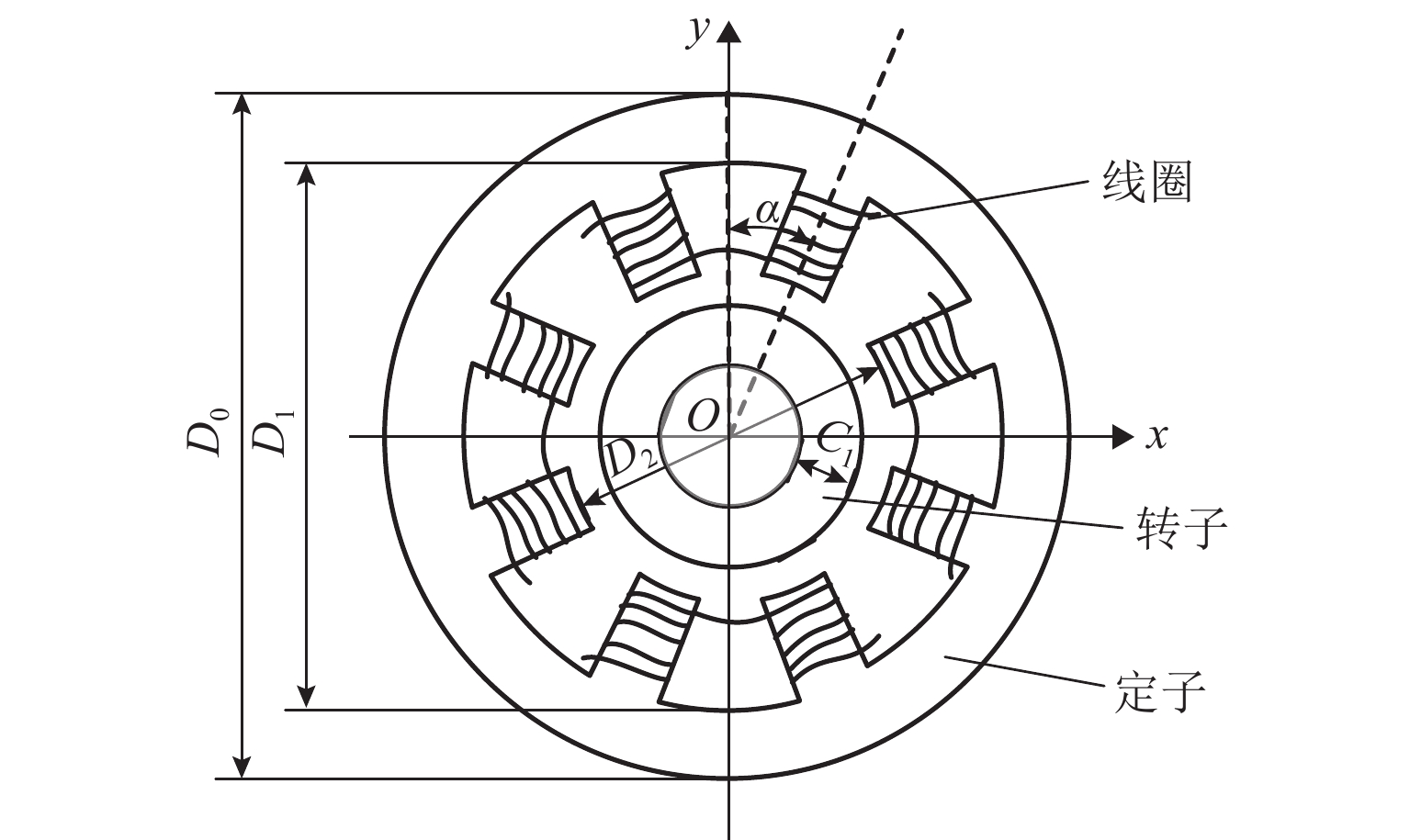
八极径向电磁阻尼器的结构如图2所示,具体参数如表1. 定子铁芯的材料为硅钢,定子由4组硅钢片组成,每组2个极,每组硅钢片上安装一组线圈.
表 1 电磁阻尼器结构参数Table 1. Structure parameters for electromagnetic damper结构参数 取值 圆盘直径 D/mm 150 转子直径 d/mm 35 阻尼器外径 D0/mm 340.6 阻尼器内径 D2/mm 153.0 定子厚度 b/mm 50 磁极面积 A/mm2 1500 线圈匝数 N/匝 280 静态电流 I0/A 2.3 磁极角 α/(°) 22.5 气隙长度 C1/mm 1.5 2.2 电磁阻尼器动态刚度
考虑涡流效应的叠片磁导率µrf表示为[16]
μrf=μrtanhλ2√jfσμ0μrλ2√jfσμ0μr, (5) 式中:µ0为真空磁导率µ0=4π × 10−7H/m;µr为静态相对磁导率,µr=5000;σ为电导率,σ=7.46 × 106 S/m;λ为叠片厚度,λ=0.35 mm;f为旋转频率.
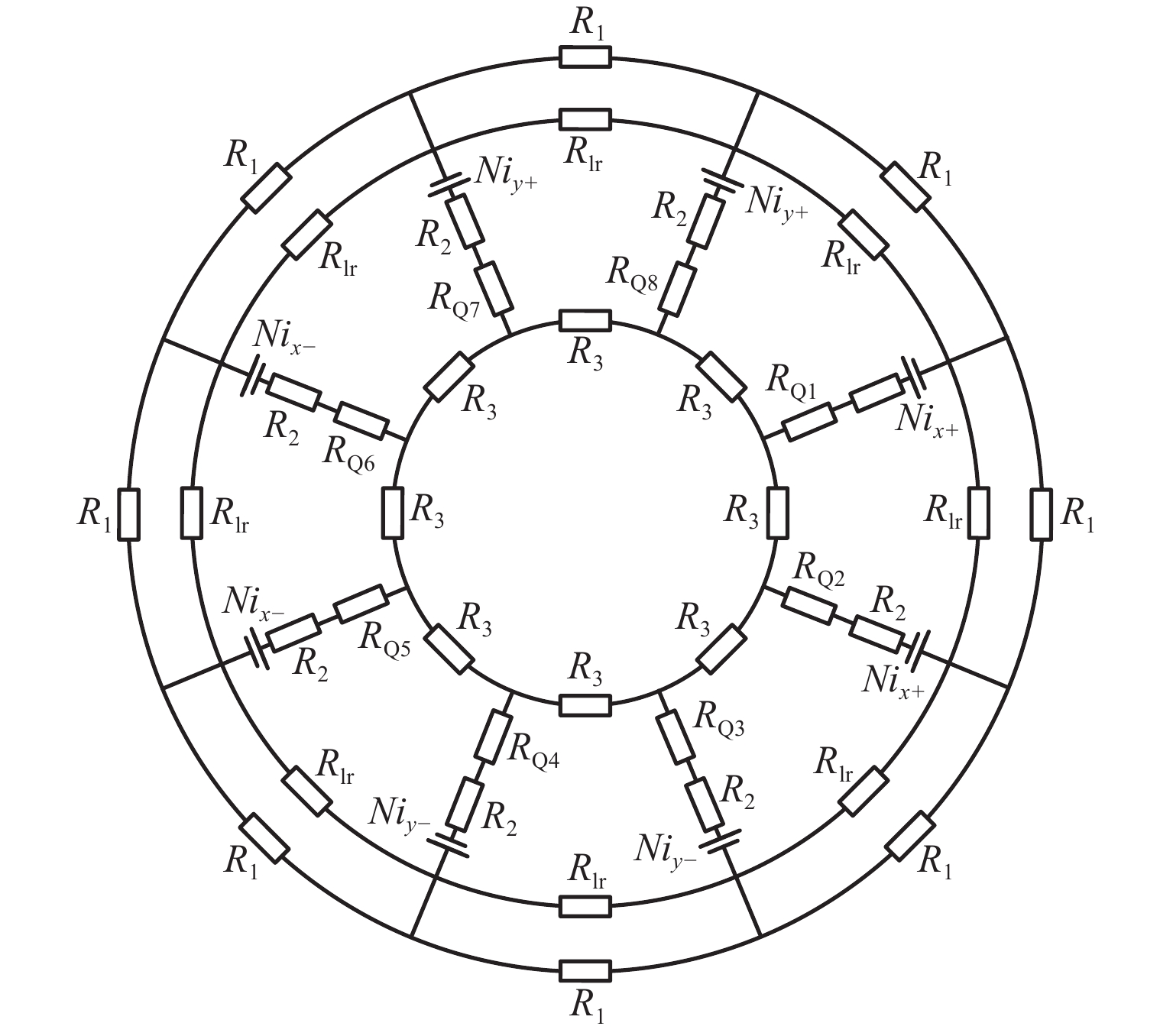
由于漏磁不可避免,考虑漏磁因素的电磁阻尼器的等效磁路模型如图3所示,图中:Rlr为漏磁阻[17-18];R1为定子磁轭动磁阻,R2为磁极动磁阻,R3为圆盘动磁阻,3种动磁阻与电磁阻尼器和圆盘的结构参数有关,其计算表达式如式(6)~(8)[17]; RQ1,RQ2,…,RQ8为气隙磁阻;iy+、iy−(ix+、ix−)分别为y+、y−(x+、x−)方向磁极线圈通入的电流.
R1=π(D0+D1)16μ0μrfb(D0−D1), (6) R2=D0+D1−2D24μ0μrfA, (7) R3=π(D+d)16μ0μrfb(D−d). (8) 为方便计算,忽略x、y方向的耦合磁通,以y方向为例,当转子沿y轴负方向(y−)移动Δy时,y+和y−方向的气隙磁阻分别为
{RQ7=RQ8=C1+Δycosαμ0A,RQ3=RQ4=C1−Δycosαμ0A. (9) 因此,y+和y−方向的总磁阻分别为
{Ry+=R1+2R2+RQ7+RQ8+R3,Ry−=R1+2R2+RQ3+RQ4+R3. (10) y+和y−方向磁极的线圈通入的电流分别为
{iy+=I0+iy,iy−=I0−iy. (11) 则转子在y方向受到的电磁合力为
Fy=2N2cosαμ0A[(I0+iy)2R2y+−(I0−iy)2R2y−], (12) 式中:iy为y方向的控制电流.
当iy较小时,式(12)可以通过平衡位置附近的一阶泰勒级数来线性表示,即:
Fy=−KΔy+Giy, (13) 式中:K为动态位移刚度,如式(14);G为动态电流刚度,如式(15).
K=−∂Fy∂Δy=8N2I02cos2αμ20A2(1R3y++1R3y−), (14) G=∂Fy∂iy=4N2I0cosαμ0A(1R2y++1R2y−). (15) 2.3 动态刚度频率响应
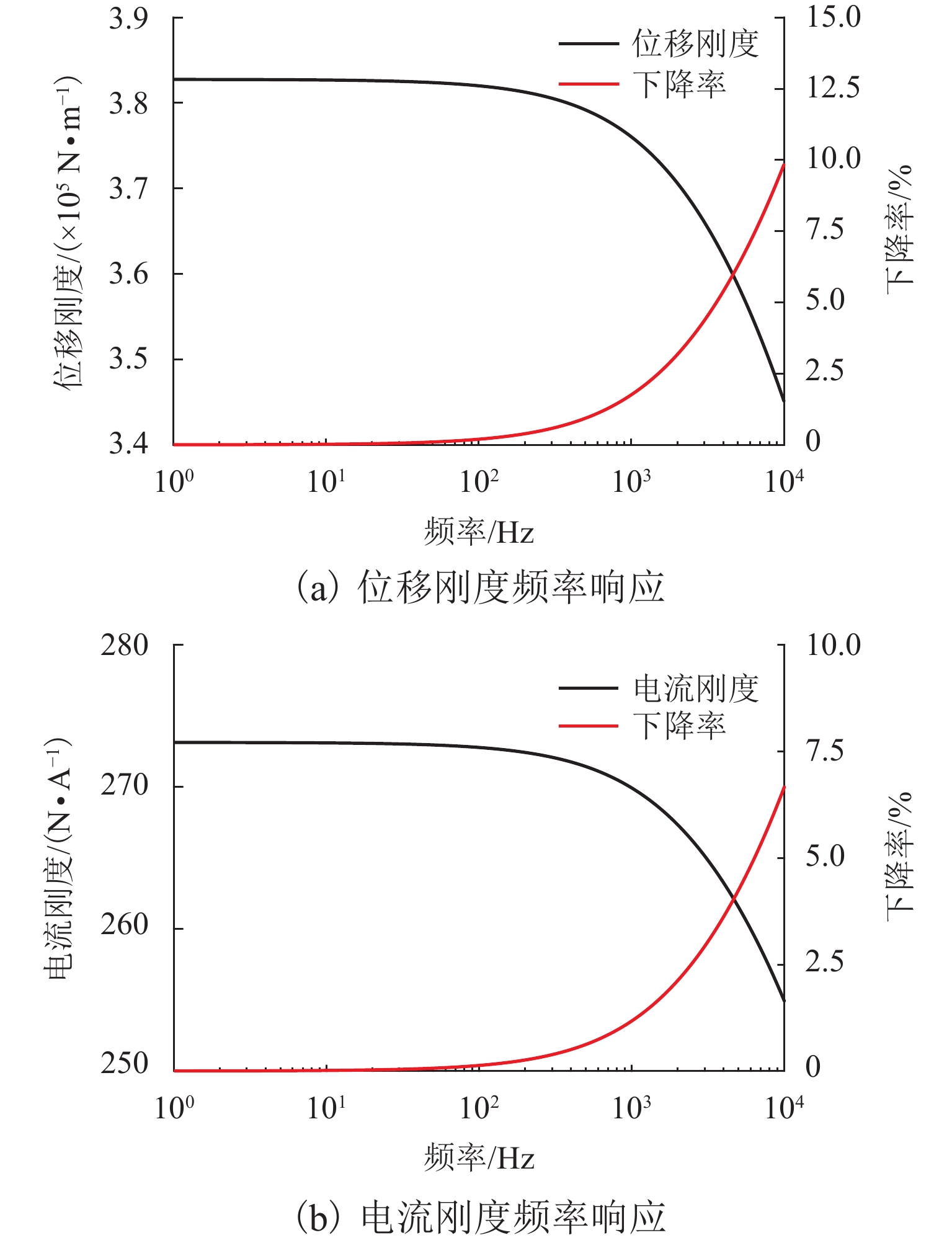
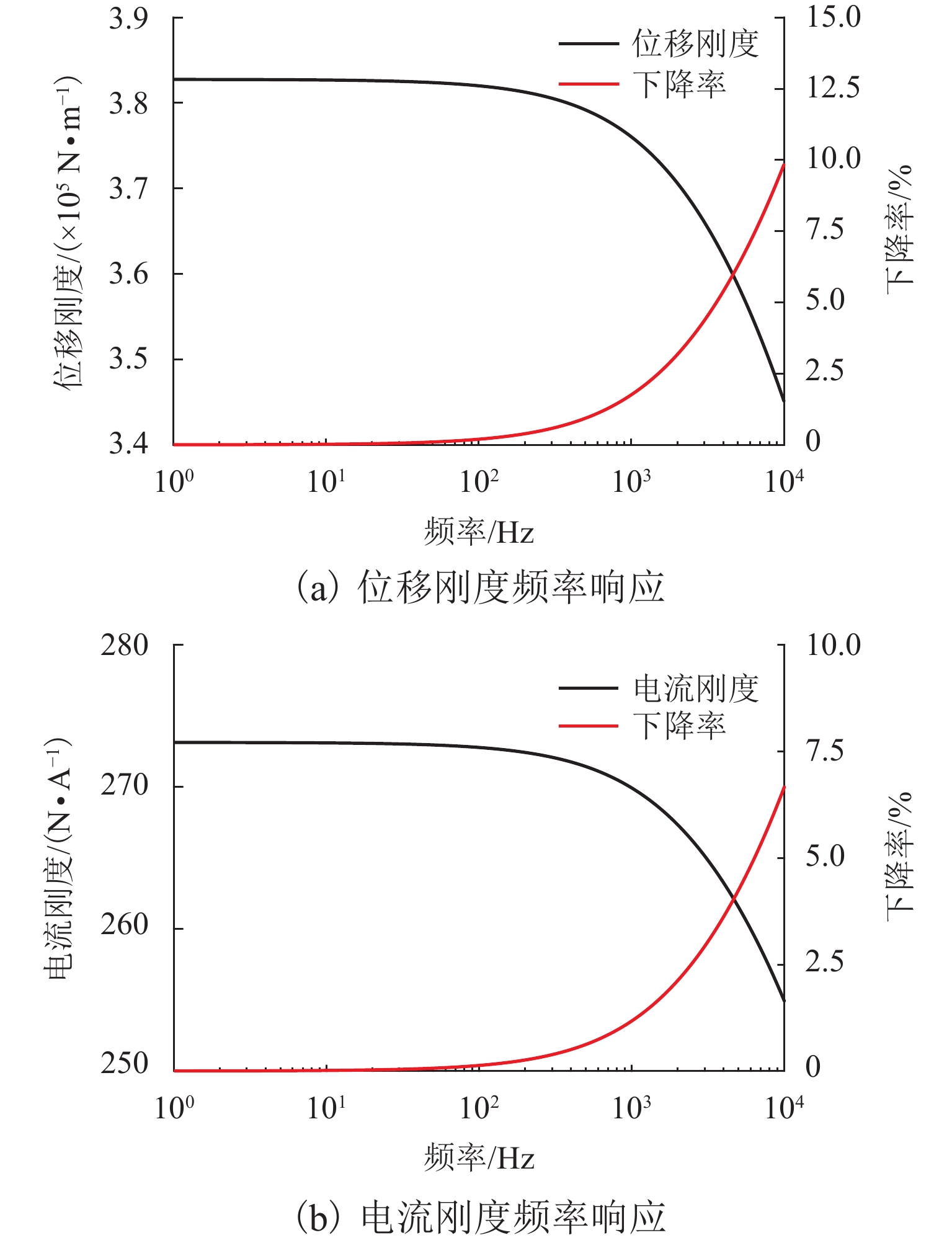
电磁阻尼器的动态刚度与旋转频率之间的关系如图4所示,可以看出,位移刚度和电流刚度随着旋转频率的增加而减小,在高速状态下表现出极其不稳定的特性,当转速为100 kHz时,理论上位移刚度和电流刚度分别下降了10.0%、6.6%,将影响电磁阻尼器的性能. 因此,为了在高速工况下提高系统的计算精度,应计及电磁阻尼器的动态刚度问题.
3. 电磁阻尼器PID参数优化
本文采用一种非线性动态自适应惯性权重PSO算法[19]对电磁阻尼器的PID的3个控制参数进行优化,该算法可自适应地赋予不同的惯性权重因子,满足粒子群优化算法在不同进化时期对全局探索和局部开发能力的需求,可避免PID控制参数陷入局部最优及初期收敛等缺点. 采取ITAE作为评判指标,适应度函数的具体表达式为
F=∫t0t|Δ(t)|dt, (16) 式中:Δ(t)为误差.
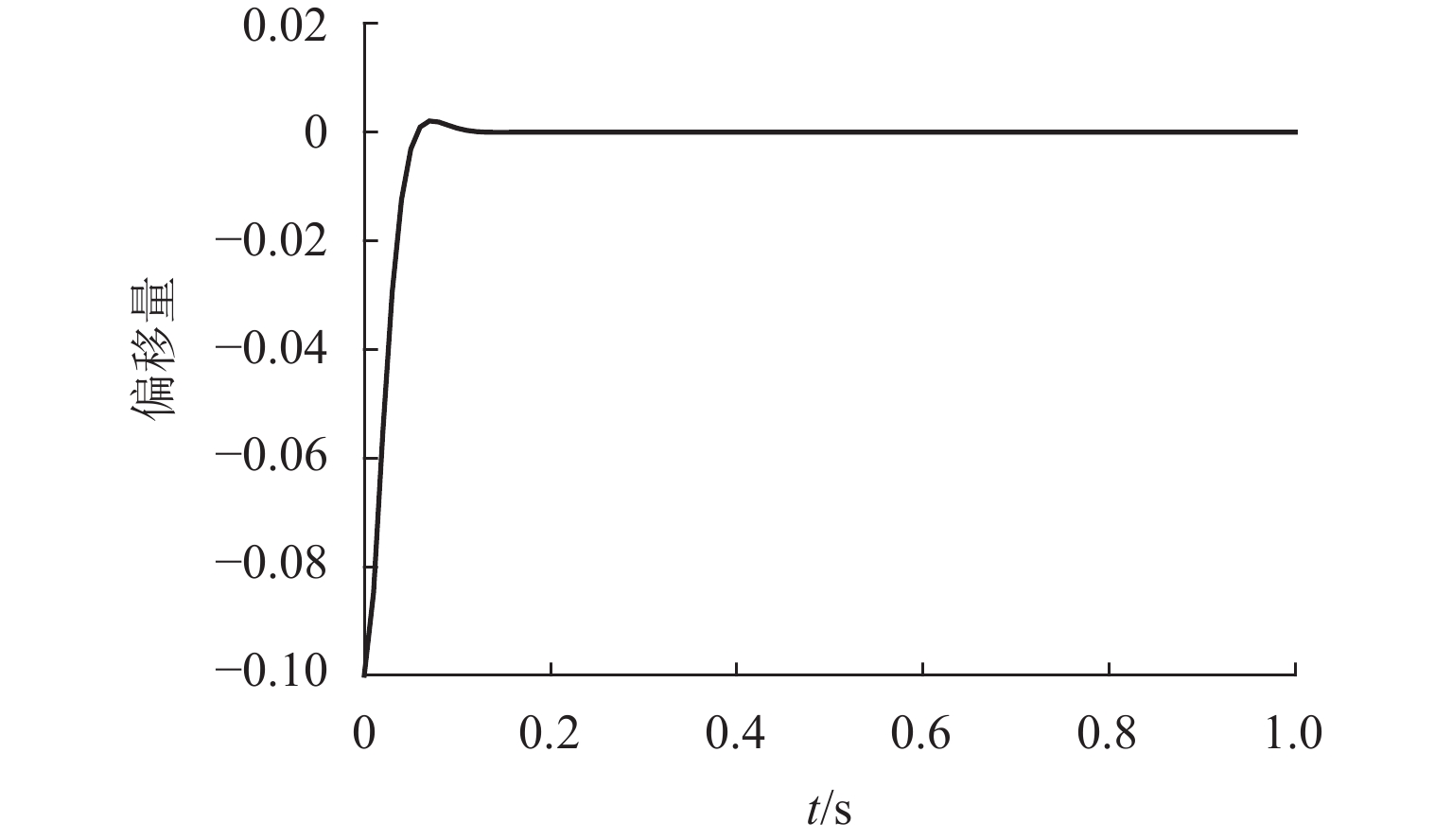
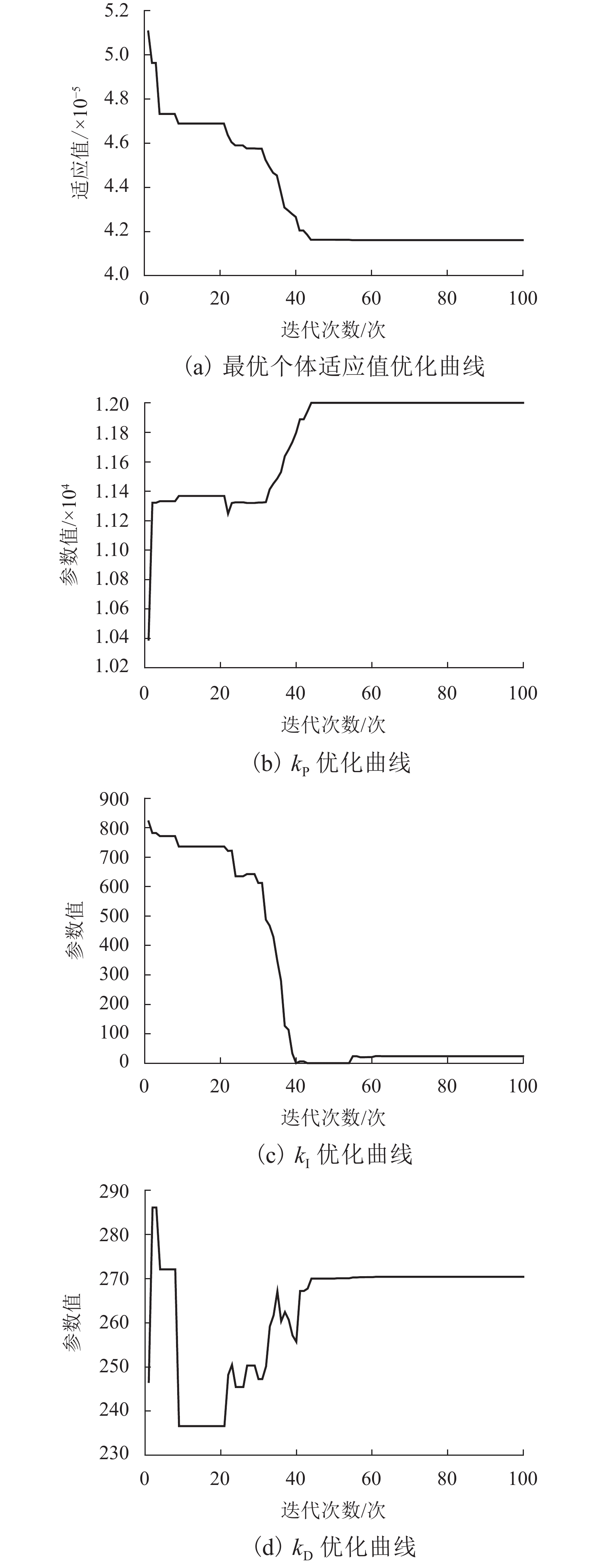
在算法优化搜寻过程中,每个粒子代表着PID控制器里待优化的一个参数集{kP,kI,kD},适应值函数达到最小值时,PID控制器的控制效果最佳. 本文粒子群规模为100,最大迭代次数为100,圆盘在x方向有初始无量纲位移, 优化曲线如图5所示.
从图5可以看出,最优个体适应值在迭代60次之后才趋于稳定,其最优适应值趋于0.000042. 此时主动电磁阻尼器PID的3个控制参数分别为kP1=12000 A/m、kI1=24 A/(m·s)、kD1=270 A·s/m.
图6为圆盘响应曲线,从图6可以看出,在该PID参数控制下,电磁阻尼器能够在0.1 s内迅速地将圆盘的偏离量调至0,并且超调量小,通过该粒子群算法得到的PID参数具有优秀的控制效果.
4. 动力学分析
在SIMULINK搭建动力学仿真模型[20],转子和电磁轴承的具体参数如表2所示.
表 2 仿真参数Table 2. Parameters of simulation系统参数 取值 圆盘质量 m1/kg 6.39 轴颈质量 m2/kg 2.15 转轴刚度 k/(N·m−1) 106 初始相位角 φ/(°) 45 原始偏心距 e0/mm 0.5 固有频率 ωn/(rad·s−1) 305 电磁轴承气隙长度 C2/mm 0.4 电磁轴承位移刚度 K2/(N·m−1) 3.657 × 105 电磁轴承电流刚度 G2/(N·A−1) 73.14 比例增益 kP2/(A·m−1) 5500 积分增益 kI2/(A·(m·s)−1) 8000 微分增益 kD2/(A·s·m−1) 3 4.1 考虑涡流效应的转子系统响应
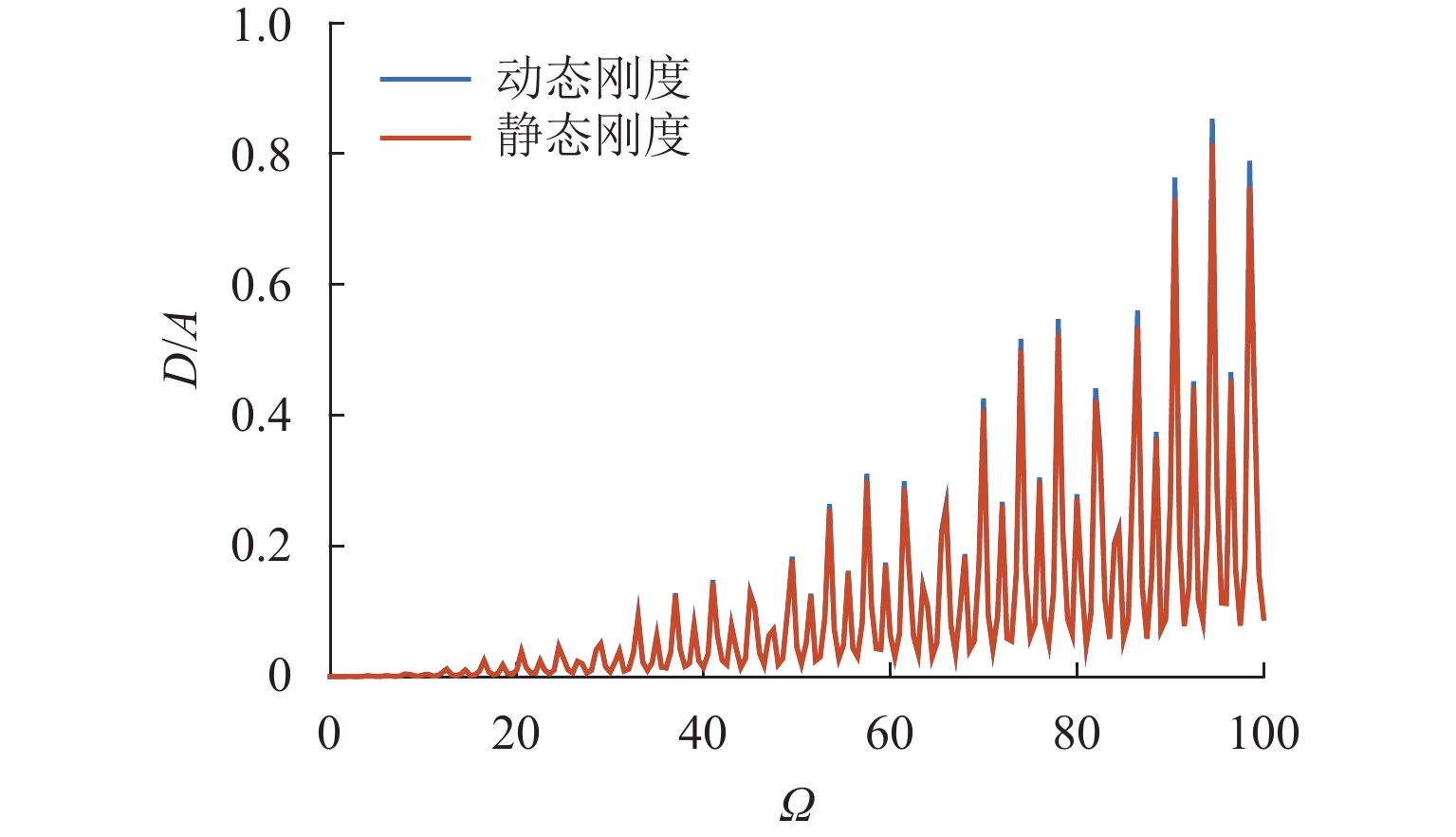
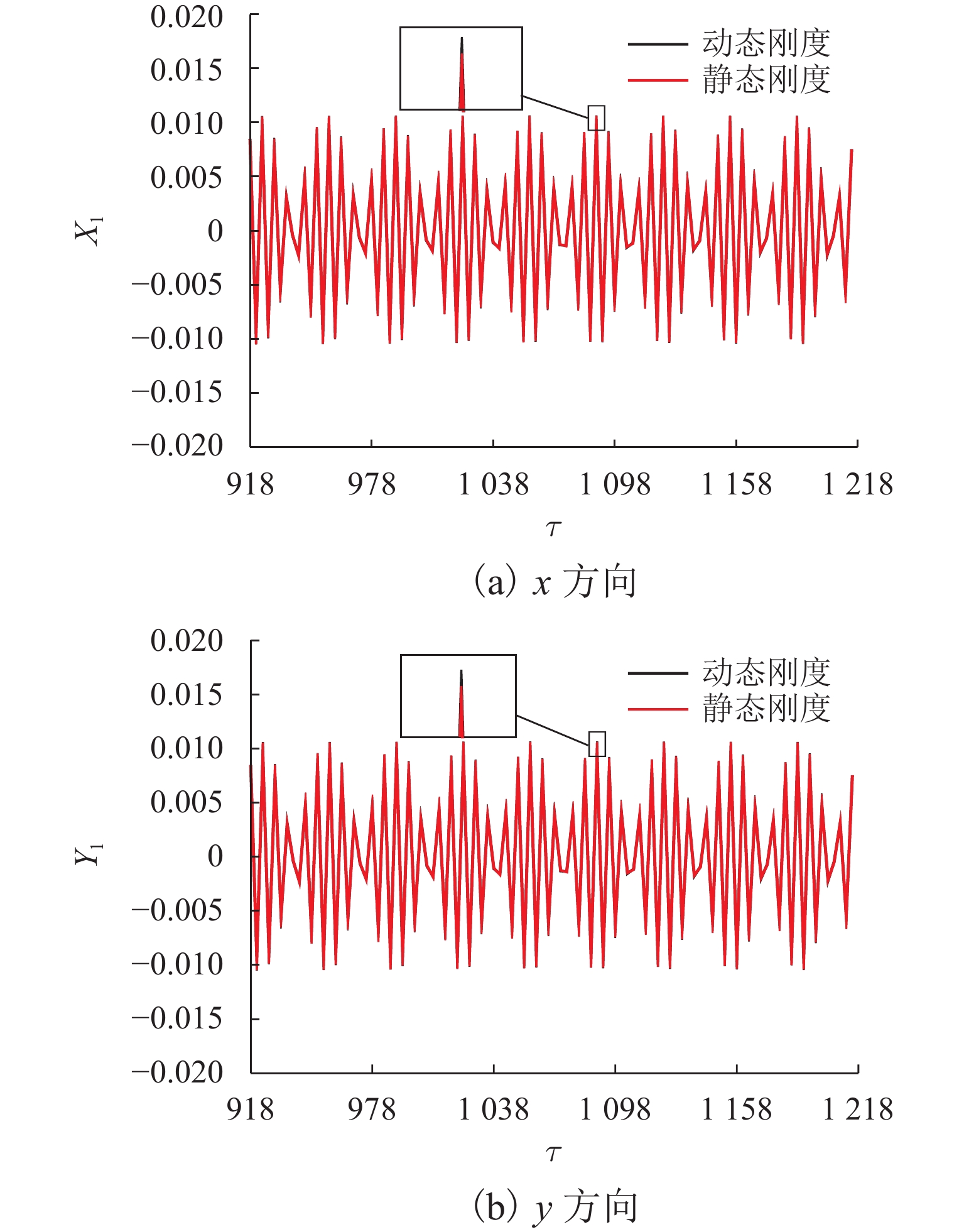
转子在高速工况下运行时,考虑涡流效应的电磁阻尼器刚度会有所削弱. 为了研究不同转速下涡流效应对转子系统响应的影响,将圆盘在动、静态刚度情况下的无量纲振幅(位移峰值与气隙长度的比值,简称D/A)进行对比,如图7所示,并计算两种刚度下无量纲振幅的相对误差,如图8所示.
从图7可以看出:两种刚度下的无量纲振幅均小于1,说明转速比Ω在[0,100.0]内,圆盘与电磁阻尼器没有发生碰撞;动态刚度下圆盘的无量纲振幅比静态刚度时的大. 由图8可知,随着转速比的增大,两种刚度下无量纲振幅的相对误差呈波动上升趋势,当转速比Ω=98.5(ω=4782 Hz),最高达到5.33%.
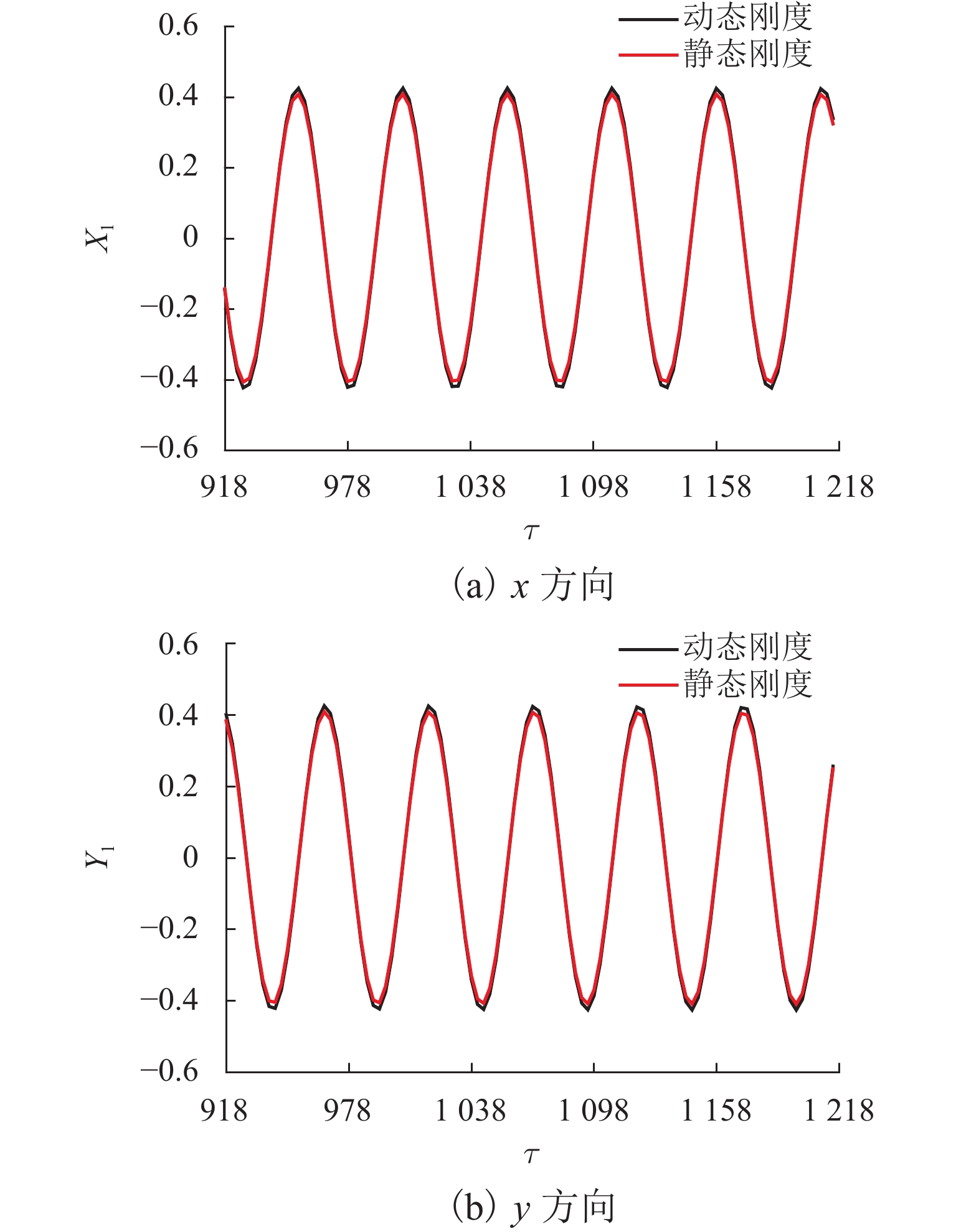
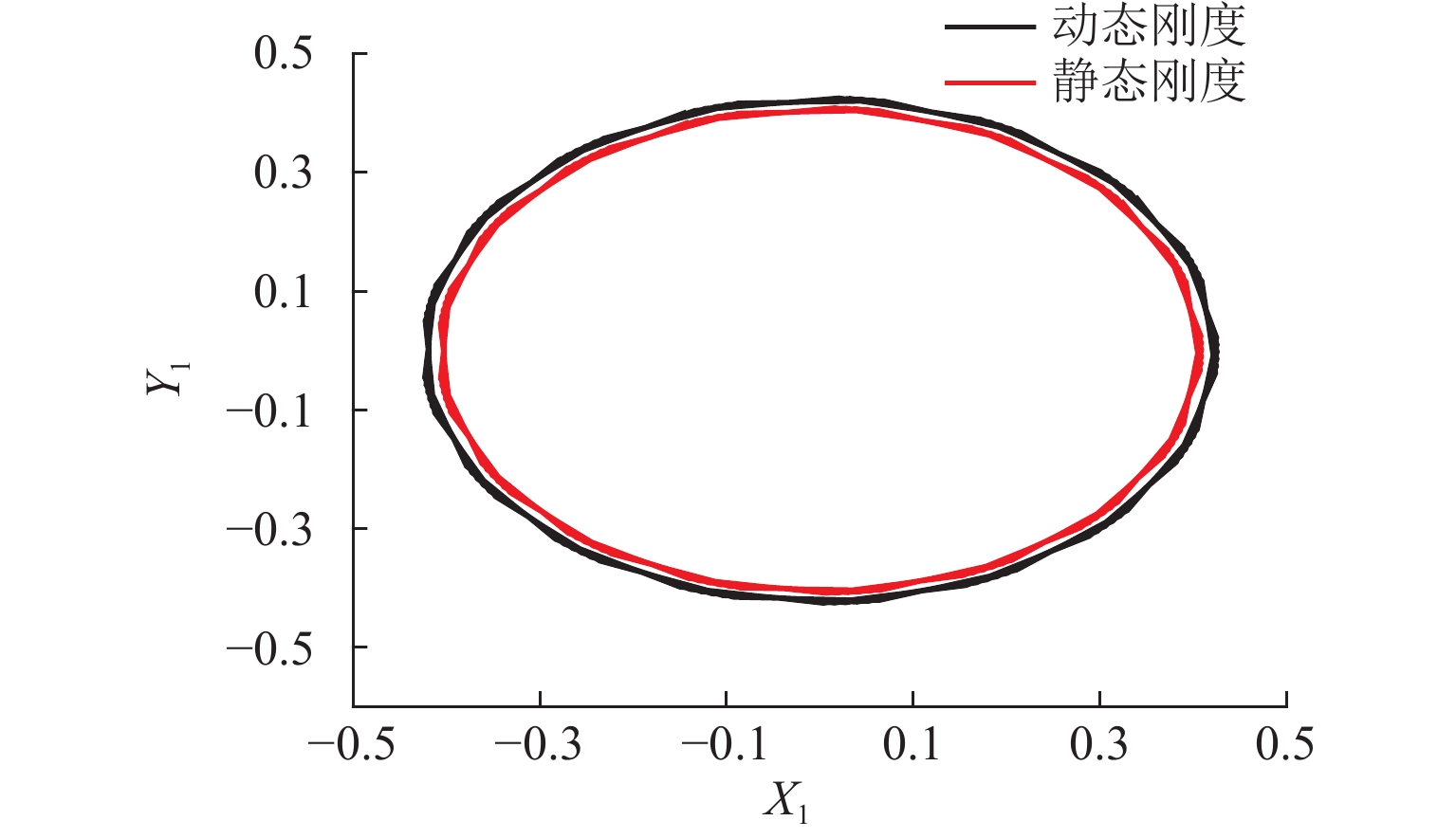
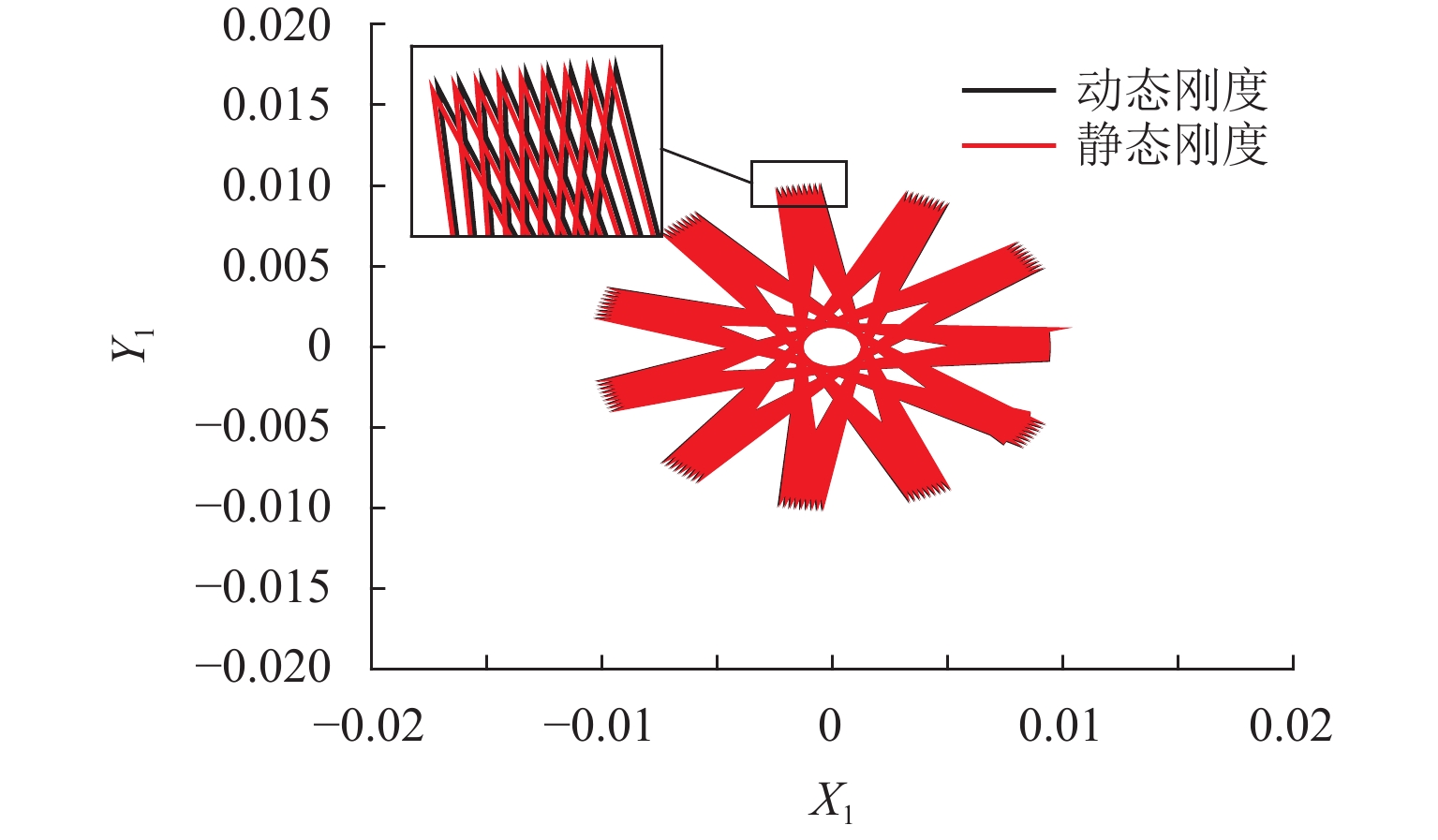
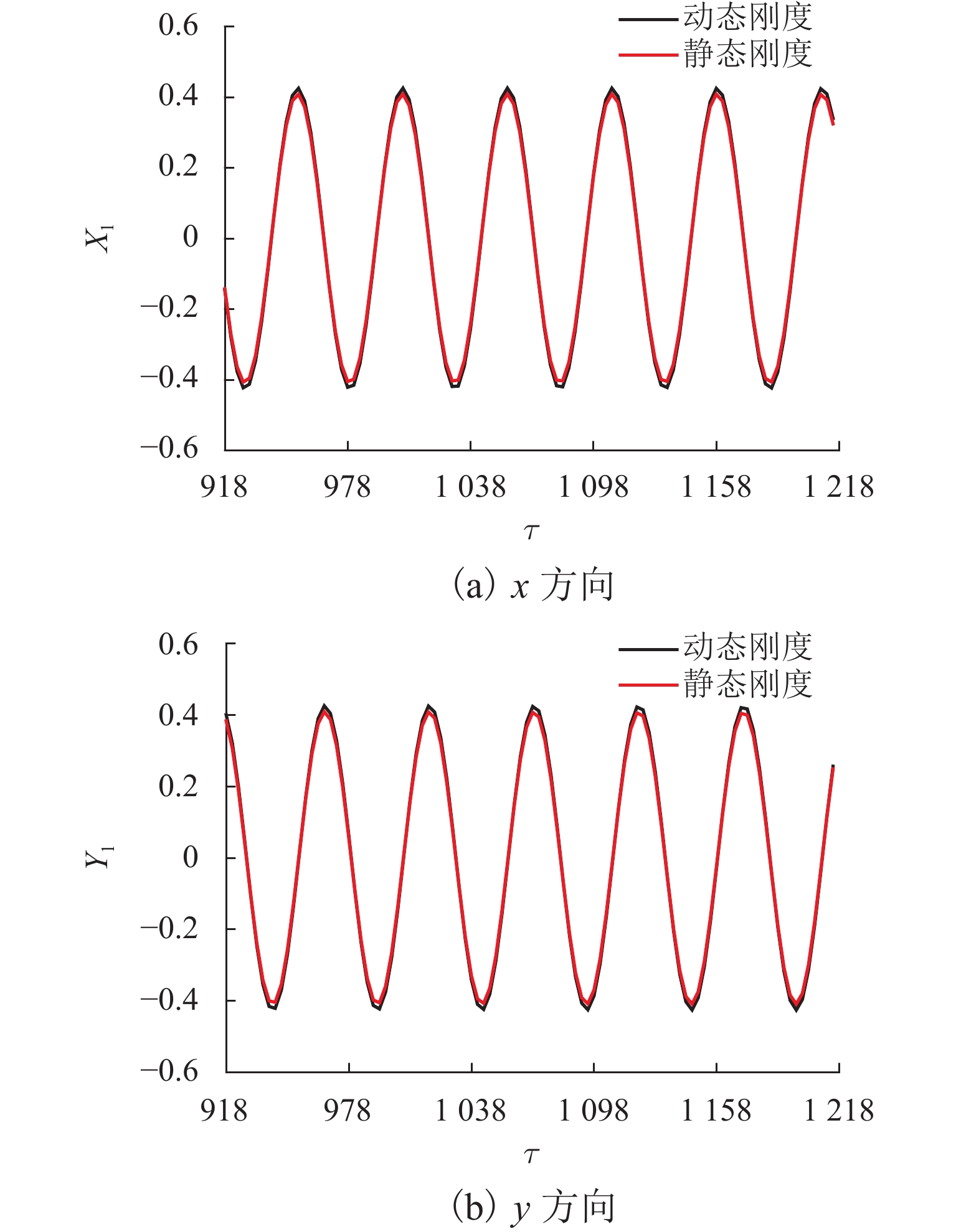
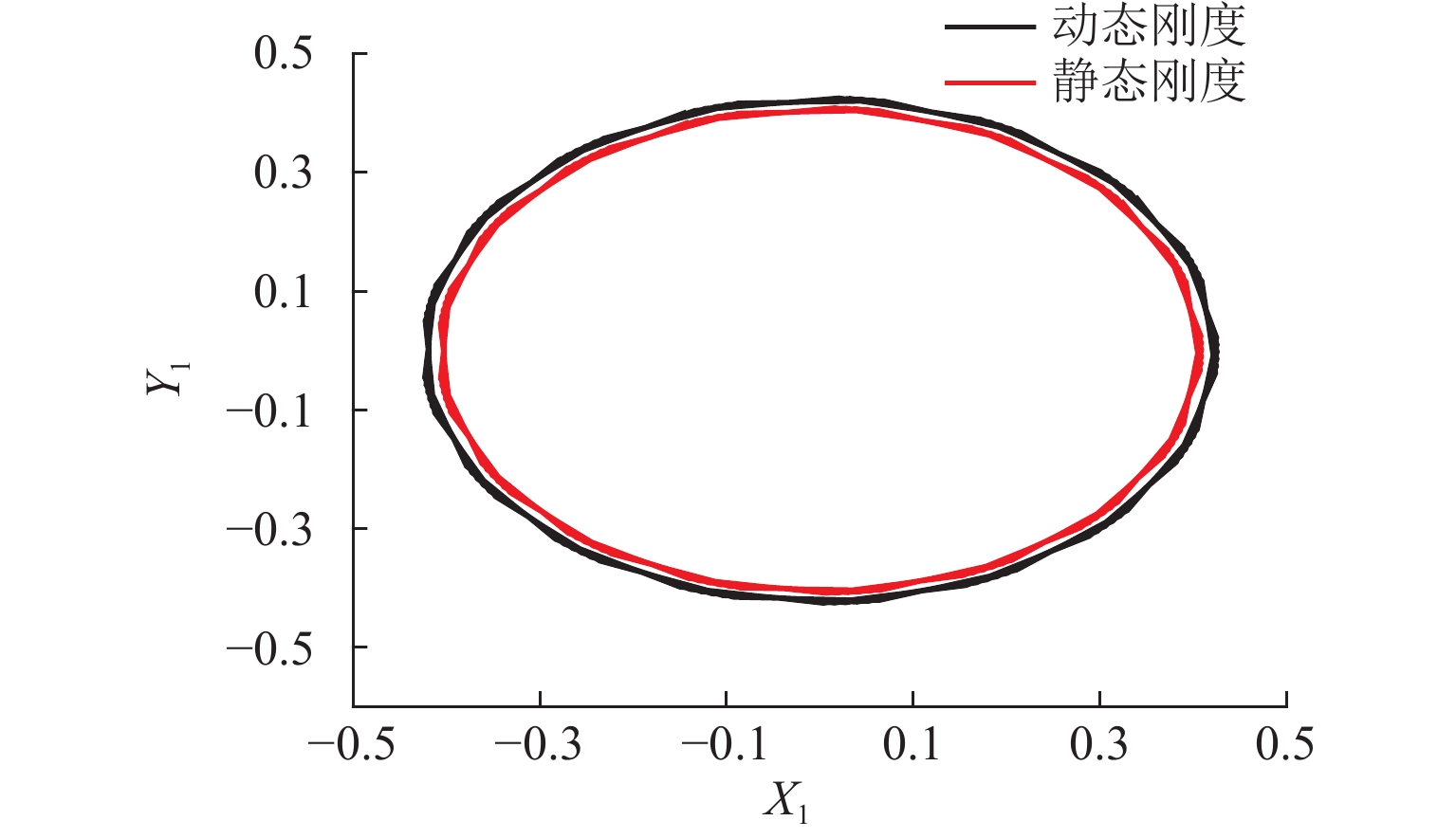
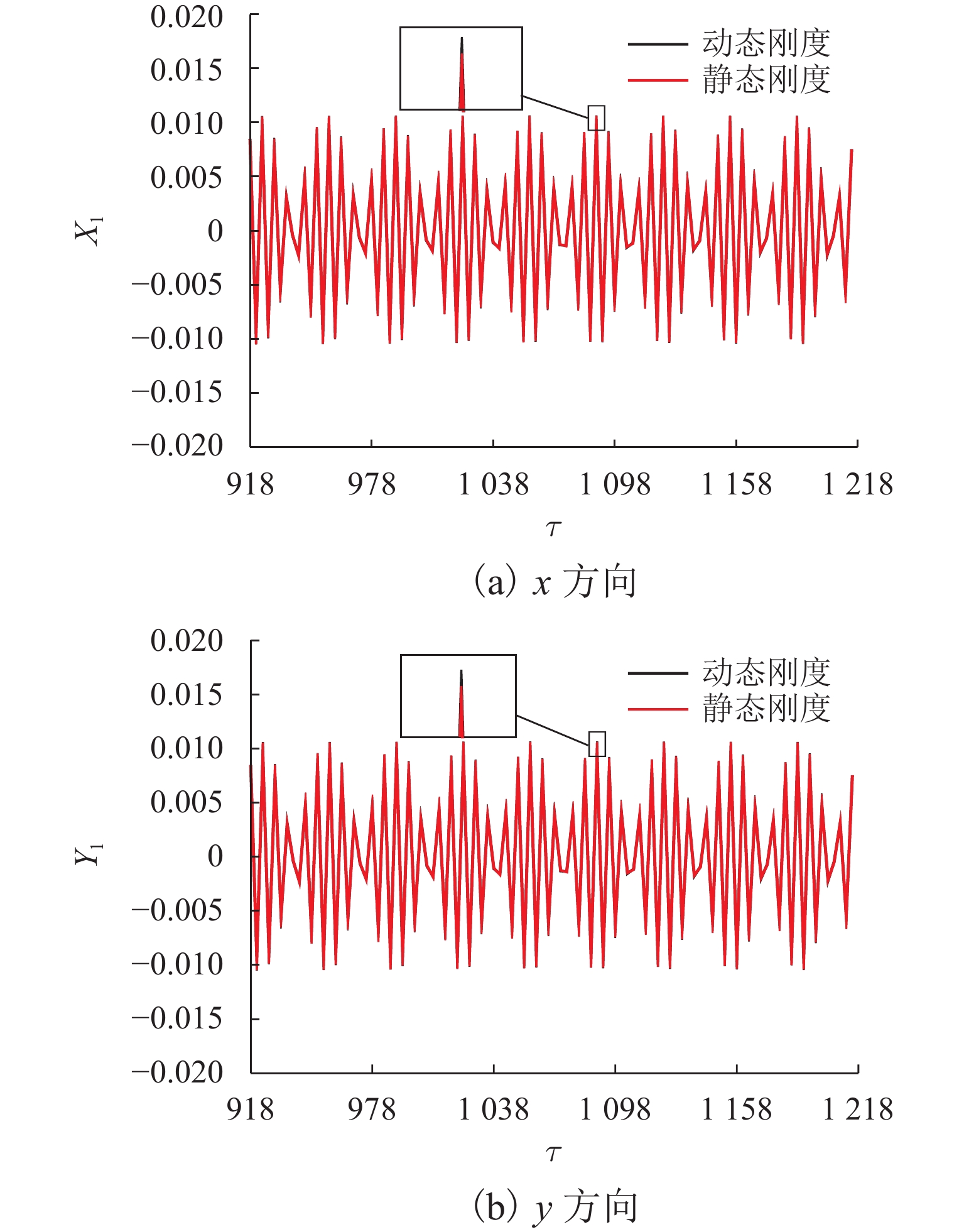
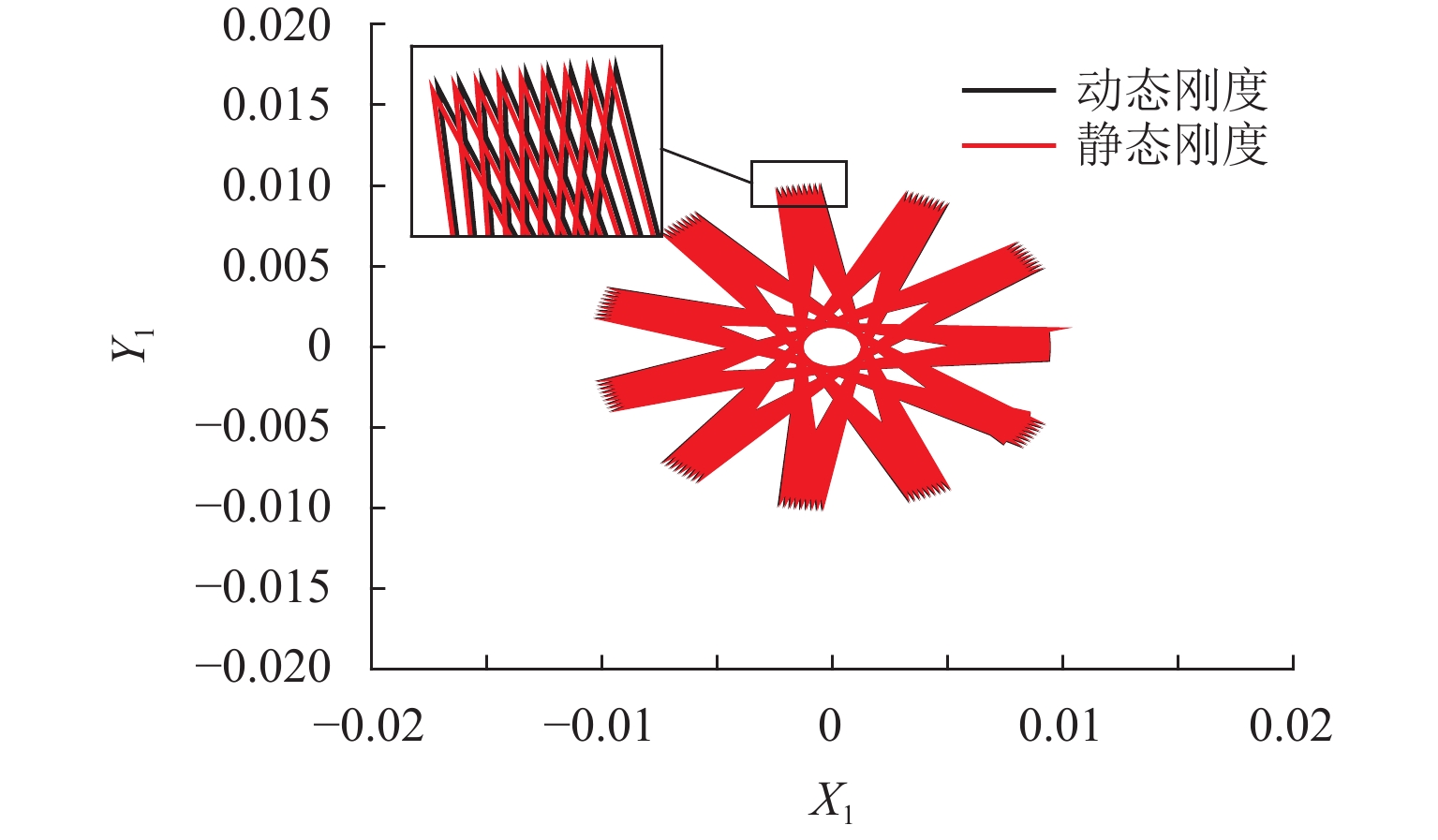
图9和图10分别为两种刚度下转速比Ω=70.0时转子系统的时域图和平稳状态下的轴心轨迹图,由于涡流效应的影响,圆盘的无量纲位移和运动轨迹范围均明显增大,这可能导致转子与密封部位接触而造成磨损. 因此,对于高速工况下转子系统,需要考虑涡流效应对电磁阻尼器的影响,通过动态刚度得到的转子系统响应更加准确.
图11和图12分别为两种刚度下转速比Ω=71.0时转子系统的时域图和平稳状态下的轴心轨迹图,对比图9、10和图11、12可以看出,Ω=70.0时,圆盘的位移要远大于Ω=71.0时圆盘的位移,并且此时涡流响应的影响更加显著,导致两种刚度下位移差值更大.
4.2 偏心距对系统响应的影响
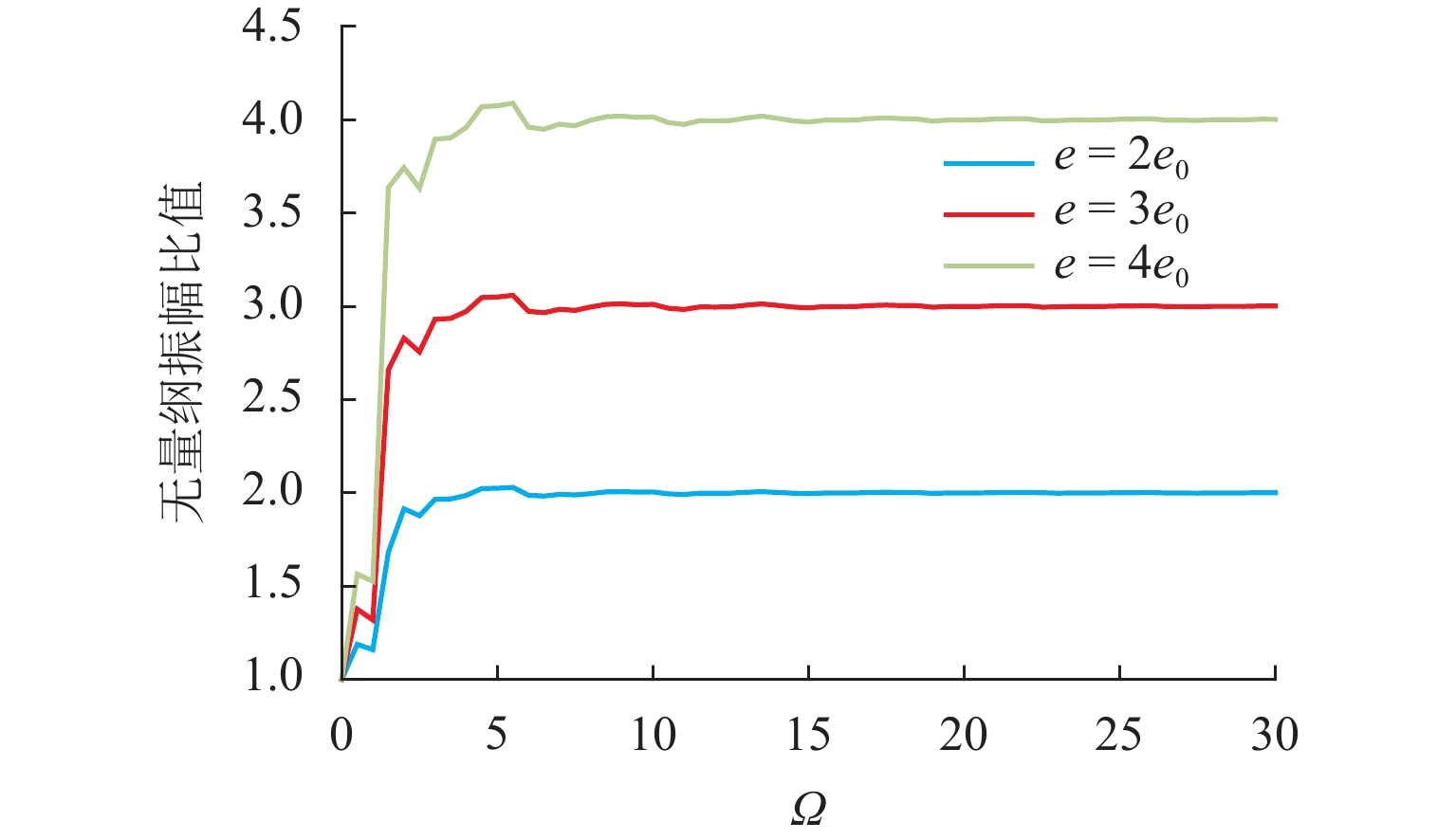
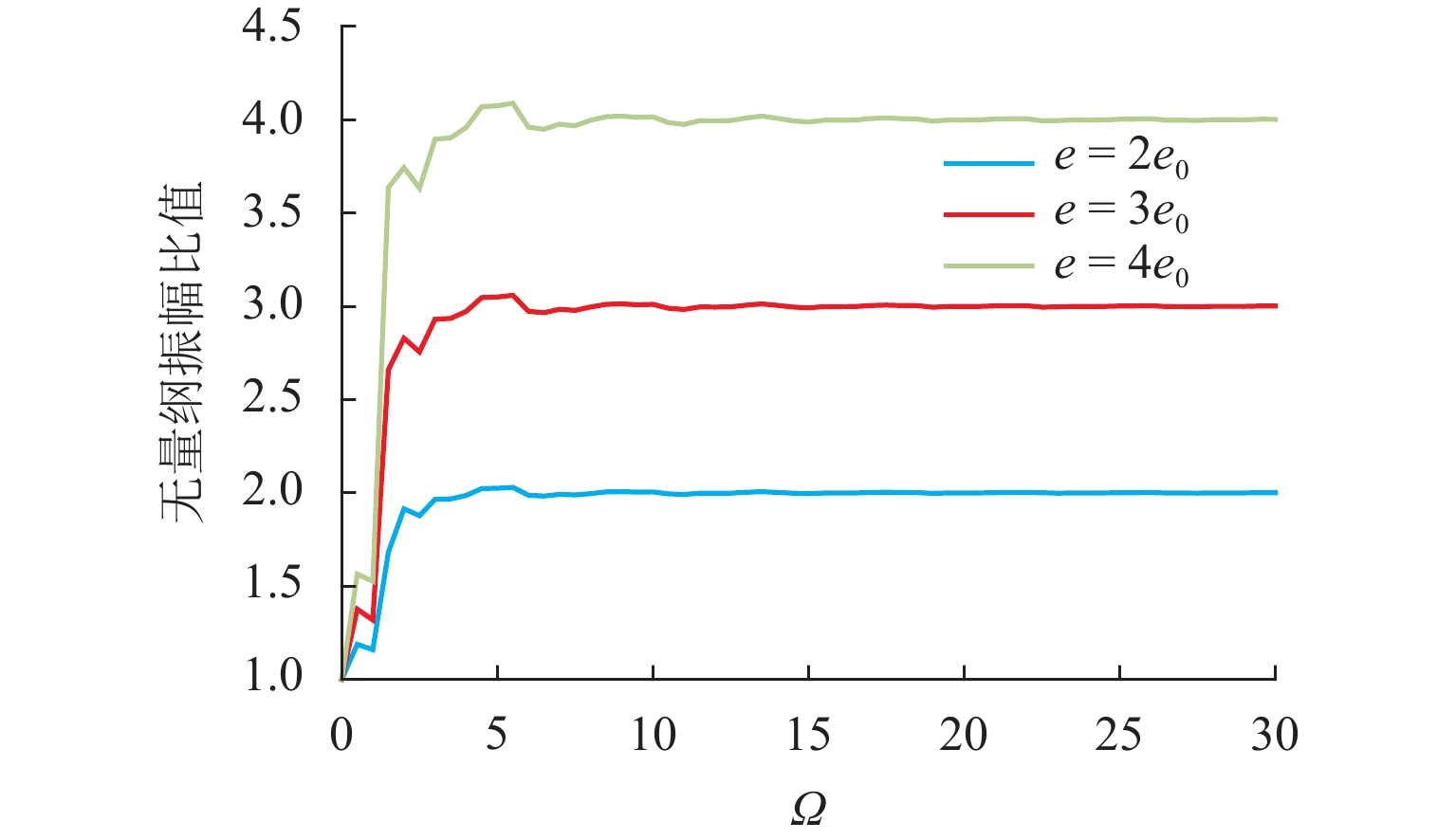
为了分析偏心距对系统响应的影响,选取偏心距分别为e0、2e0、3e0和4e0时,圆盘在不同转速比下的无量纲振幅对比分析,如图13. 并且将后3种偏心距下圆盘的无量纲振幅除以原始偏心距下圆盘的无量纲振幅,如图14所示.
从图13、14可以看出:当转子转速较小(Ω≤1.5,ω≤73 Hz)时,随着偏心距的增大,圆盘的无量纲振幅增大幅度较小;当转子转速不断变大,到达较高转速(Ω≥5.0,ω≥242 Hz)时,无量纲振幅比值不再变化,此后,随着偏心距的增大,圆盘的无量纲振幅增大幅度较大,且无量纲振幅增大的幅度与圆盘偏心距增大的幅度几乎成正比. 因此,对于高速工况下的转子系统,应尽可能地减小偏心距.
5. 结 论
本文采用解析法对电磁阻尼器的动态刚度进行了分析,并研究了带有电磁阻尼器的电磁轴承-转子系统的动力学特性. 具体结论如下:
1) 推导了涡流及漏磁效应下电磁阻尼器的动态刚度的解析模型,并定量地给出了动态刚度的变化规律. 结果表明,当转速为100 kHz时,位移刚度幅值和电流刚度幅值分别下降了10.0%、6.6%.
2) 采用一种非线性动态自适应惯性权重 PSO算法对电磁阻尼器的PID的3个控制参数进行了优化,结果表明该算法得到的PID参数具有优秀的控制效果.
3) 建立了带有电磁阻尼器的电磁轴承-转子系统的动力学模型并对其数值仿真. 结果表明,涡流效应会使圆盘的位移增大,与Ω=71.0相比,Ω=70.0时系统涡流响应的影响更加显著. 当转速为4782 Hz时,圆盘的振幅升高了5.33%. 偏心距增大会导致圆盘的振幅增大,当转速超过242 Hz时,圆盘振幅增大的幅度与圆盘偏心距增大的幅度几乎成正比.
-
表 1 电磁阻尼器结构参数
Table 1. Structure parameters for electromagnetic damper
结构参数 取值 圆盘直径 D/mm 150 转子直径 d/mm 35 阻尼器外径 D0/mm 340.6 阻尼器内径 D2/mm 153.0 定子厚度 b/mm 50 磁极面积 A/mm2 1500 线圈匝数 N/匝 280 静态电流 I0/A 2.3 磁极角 α/(°) 22.5 气隙长度 C1/mm 1.5 表 2 仿真参数
Table 2. Parameters of simulation
系统参数 取值 圆盘质量 m1/kg 6.39 轴颈质量 m2/kg 2.15 转轴刚度 k/(N·m−1) 106 初始相位角 φ/(°) 45 原始偏心距 e0/mm 0.5 固有频率 ωn/(rad·s−1) 305 电磁轴承气隙长度 C2/mm 0.4 电磁轴承位移刚度 K2/(N·m−1) 3.657 × 105 电磁轴承电流刚度 G2/(N·A−1) 73.14 比例增益 kP2/(A·m−1) 5500 积分增益 kI2/(A·(m·s)−1) 8000 微分增益 kD2/(A·s·m−1) 3 -
[1] 张凤阁,杜光辉,王天煜,等. 高速电机发展与设计综述[J]. 电工技术学报,2016,31(7): 1-18. doi: 10.3969/j.issn.1000-6753.2016.07.001ZHANG Fengge, DU Guanghui, WANG Tianyu, et al. Review on development and design of high speed machines[J]. Transactions of China Electrotechnical Society, 2016, 31(7): 1-18. doi: 10.3969/j.issn.1000-6753.2016.07.001 [2] ZHANG Y P, HE L D, YANG J J, et al. Vibration control of Tie rod rotors with optimization of unbalanced force and unbalanced moment[J]. IEEE Access, 2020, 8: 66578-66587. doi: 10.1109/ACCESS.2020.2985847 [3] HEINDEL S, MÜLLER P C, RINDERKNECHT S. Unbalance and resonance elimination with active bearings on general rotors[J]. Journal of Sound and Vibration, 2018, 431: 422-440. doi: 10.1016/j.jsv.2017.07.048 [4] 吴华春,涂星,周建,等. 磁悬浮转子不平衡振动控制研究综述[J]. 轴承,2022(3): 1-9. doi: 10.19533/j.issn1000-3762.2022.03.001WU Huachun, TU Xing, ZHOU Jian, et al. Review on unbalanced vibration control for magnetic suspension rotor[J]. Bearing, 2022(3): 1-9. doi: 10.19533/j.issn1000-3762.2022.03.001 [5] 黄威,邓智泉,李克翔,等. 一种磁悬浮轴承支承刚性转子现场动平衡方法[J]. 电工技术学报,2020,35(22): 4636-4646. doi: 10.19595/j.cnki.1000-6753.tces.191289HUANG Wei, DENG Zhiquan, LI Kexiang, et al. A filed dynamic balancing method for rigid rotor supported by magnetic bearings[J]. Transactions of China Electrotechnical Society, 2020, 35(22): 4636-4646. doi: 10.19595/j.cnki.1000-6753.tces.191289 [6] SHE C F, ZHANG M, GE Y B, et al. Design and simulation analysis of an electromagnetic damper for reducing shimmy in electrically actuated nose wheel steering systems[J]. Aerospace, 2022, 9(2): 1-21. doi: 10.3390/aerospace9020113 [7] 黄翠翠,李晓龙,杨洋,等. 基于自抗扰技术的机械-电磁悬浮复合隔振控制[J]. 西南交通大学学报,2022,57(3): 582-587,617. doi: 10.3969/j.issn.0258-2724.20210850HUANG Cuicui, LI Xiaolong, YANG Yang, et al. Mechanical-electromagnetic suspension compound vibration isolation control based on active disturbance rejection technology[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 582-587,617. doi: 10.3969/j.issn.0258-2724.20210850 [8] CAI Q L, HUA Y Y, ZHU S Y. Energy-harvesting adaptive vibration damping in high-speed train suspension using electromagnetic dampers[J]. International Journal of Structural Stability and Dynamics, 2021, 21(14): 2140002.1-2140002.28. [9] XIA X J, ZHENG M Y, LIU P F, et al. Friction observer-based hybrid controller for a seat suspension with semi-active electromagnetic damper[J]. Mechatronics, 2021, 76: 102568.1-102568.11. [10] KAVIANIPOUR O. Effects of the passive electromagnetic damper on the behavior of a fluid-conveying pipeline[J]. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 2019, 233(7): 2329-2339. doi: 10.1177/0954406218784627 [11] 祝长生,钟志贤. 带主动电磁阻尼器的裂纹转子系统动力学[J]. 振动工程学报,2010,23(3): 298-304. doi: 10.3969/j.issn.1004-4523.2010.03.010ZHU Changsheng, ZHONG Zhixian. The dynamics of a cracked rotor with active magnetic dampers[J]. Journal of Vibration Engineering, 2010, 23(3): 298-304. doi: 10.3969/j.issn.1004-4523.2010.03.010 [12] ZACCARDO V M, BUCKNER G D. Active magnetic dampers for controlling lateral rotor vibration in high-speed rotating shafts[J]. Mechanical Systems and Signal Processing, 2021, 152: 107445.1-107445.16. [13] XIANG B, WONG W. Electromagnetic vibration absorber for torsional vibration in high speed rotational machine[J]. Mechanical Systems and Signal Processing, 2020, 140: 106639.1-106639.18. [14] SUN J J, ZHOU H, JU Z Y. Dynamic stiffness analysis and measurement of radial active magnetic bearing in magnetically suspended molecular pump[J]. Scientific Reports, 2020, 10: 1401.1-1401.16. doi: 10.1038/s41598-020-70784-7 [15] SUN J J, ZHOU H, MA X, et al. Study on PID tuning strategy based on dynamic stiffness for radial active magnetic bearing[J]. ISA Transactions, 2018, 80: 458-474. doi: 10.1016/j.isatra.2018.07.036 [16] LE Y, FANG J C, HAN B C, et al. Dynamic circuit model of a radial magnetic bearing with permanent magnet bias and laminated cores[J]. International Journal of Applied Electromagnetics and Mechanics, 2014, 46(1): 43-60. doi: 10.3233/JAE-141746 [17] 肖玲,赵晨曦,窦经纬,等. 轴-径向混合磁轴承动态特性及控制研究[J]. 西南交通大学学报,2022,57(3): 640-647,656. doi: 10.3969/j.issn.0258-2724.20210883XIAO Ling, ZHAO Chenxi, DOU Jingwei, et al. Research on dynamic characteristics and control of axial-radial hybrid magnetic bearing[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 640-647,656. doi: 10.3969/j.issn.0258-2724.20210883 [18] XIAO L, HE X W, CHENG W J, et al. Structural optimization and dynamic characteristics of the new type 3-degrees of freedom axial and radial hybrid magnetic bearing[J]. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 2022, 236(9): 5097-5110. doi: 10.1177/09544062211052826 [19] 王生亮,刘根友. 一种非线性动态自适应惯性权重PSO算法[J]. 计算机仿真,2021,38(4): 249-253,451. doi: 10.3969/j.issn.1006-9348.2021.04.050WANG Shengliang, LIU Genyou. A nonlinear dynamic adaptive inertial weight particle swarm optimization[J]. Computer Simulation, 2021, 38(4): 249-253,451. doi: 10.3969/j.issn.1006-9348.2021.04.050 [20] KUMAR P, TIWARI R. Dynamic analysis and identification of unbalance and misalignment in a rigid rotor with two offset discs levitated by active magnetic bearings: a novel trial misalignment approach[J]. Propulsion and Power Research, 2021, 10(1): 58-82. doi: 10.1016/j.jppr.2020.06.003 期刊类型引用(1)
1. 何少东,唐长亮,徐静雯,宋锦华,木孟良. 柔性支承的立式转子主动电磁阻尼减振研究. 电子测量技术. 2025(05): 22-29 .  百度学术
百度学术其他类型引用(2)
-






 下载:
下载:














 下载:
下载:





 百度学术
百度学术