Theoretical Study on Uplift Calculation of Embedded Twelve Ground Screws of Transmission Angle Steel Tower
-
摘要:
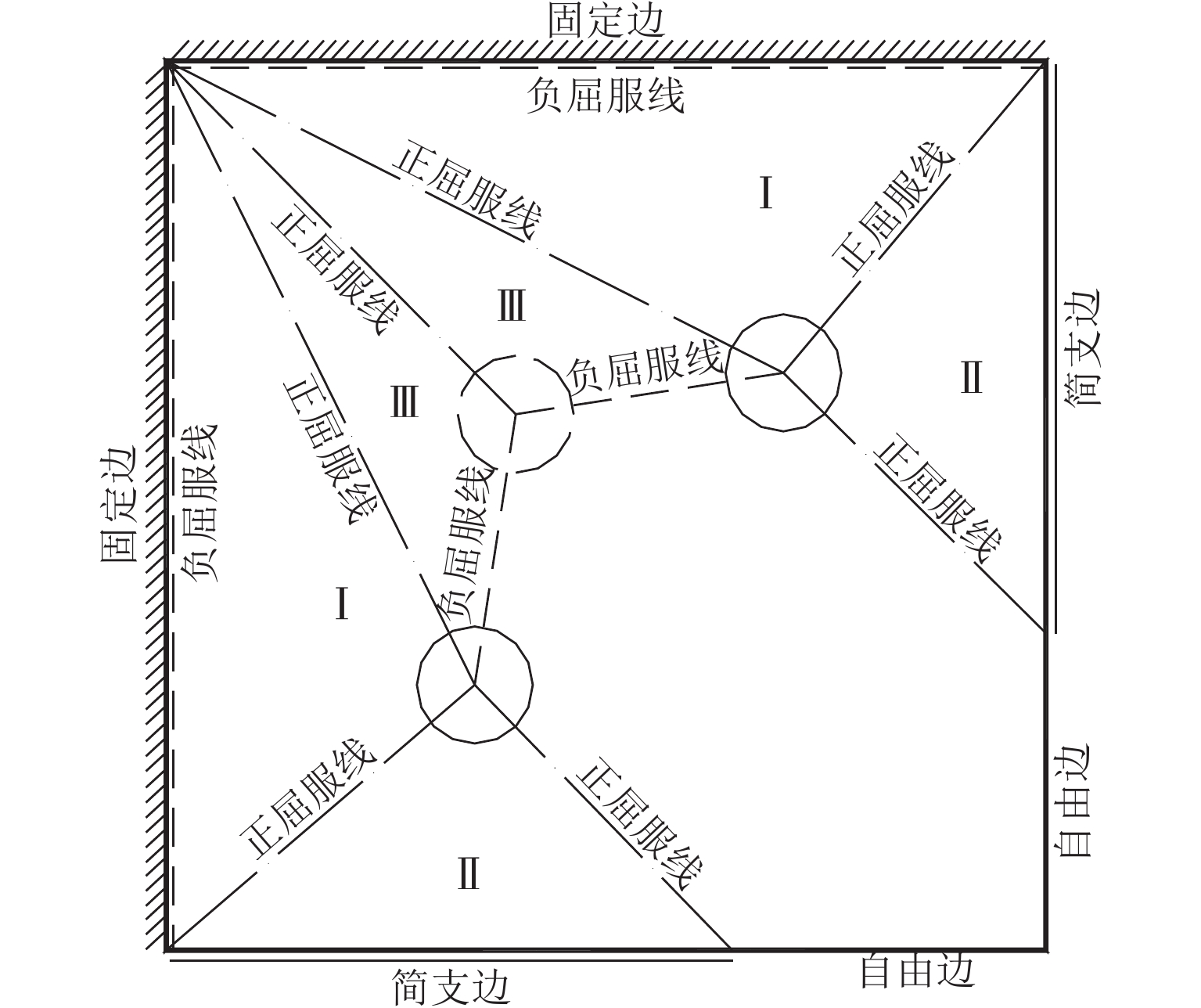
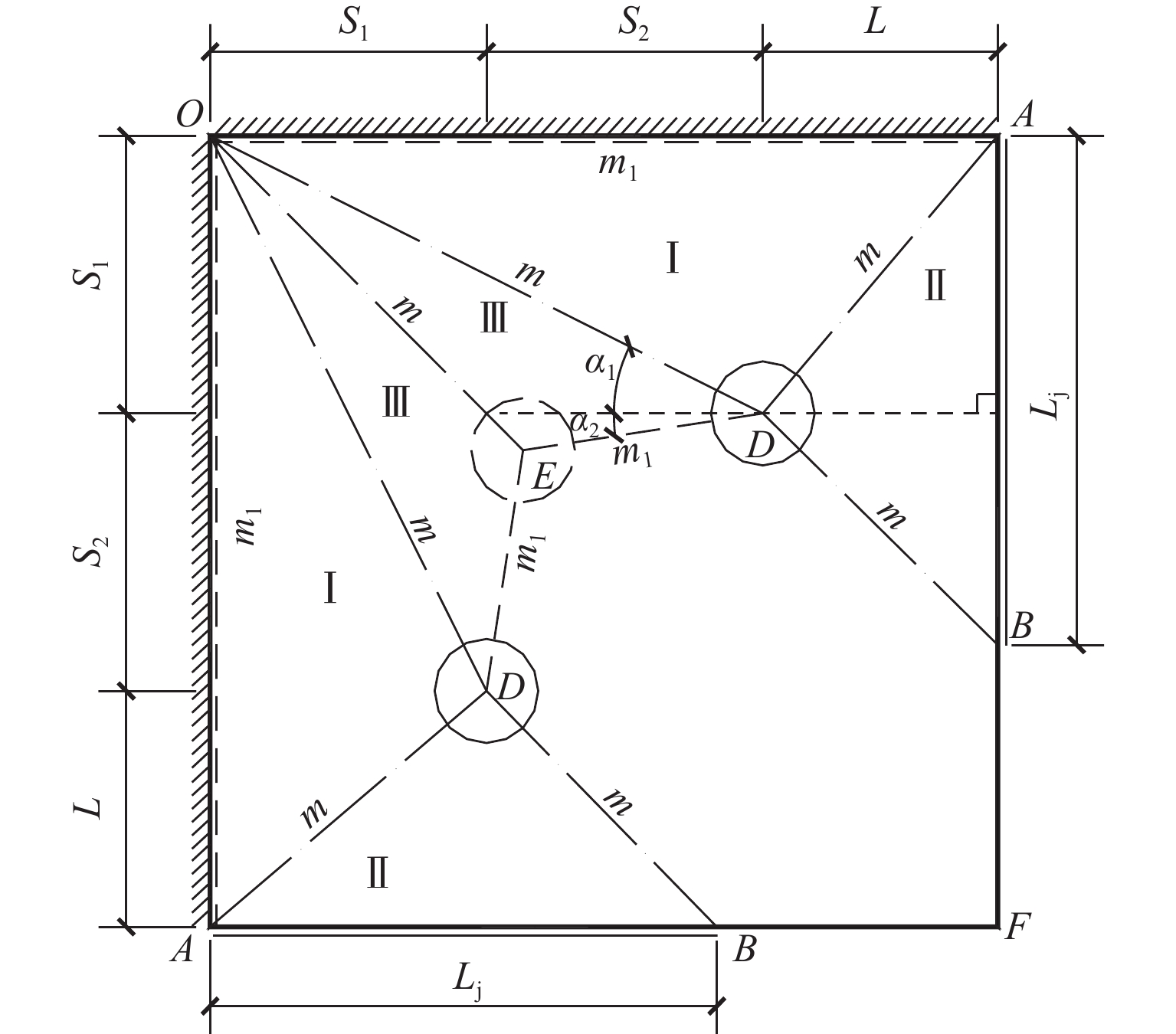
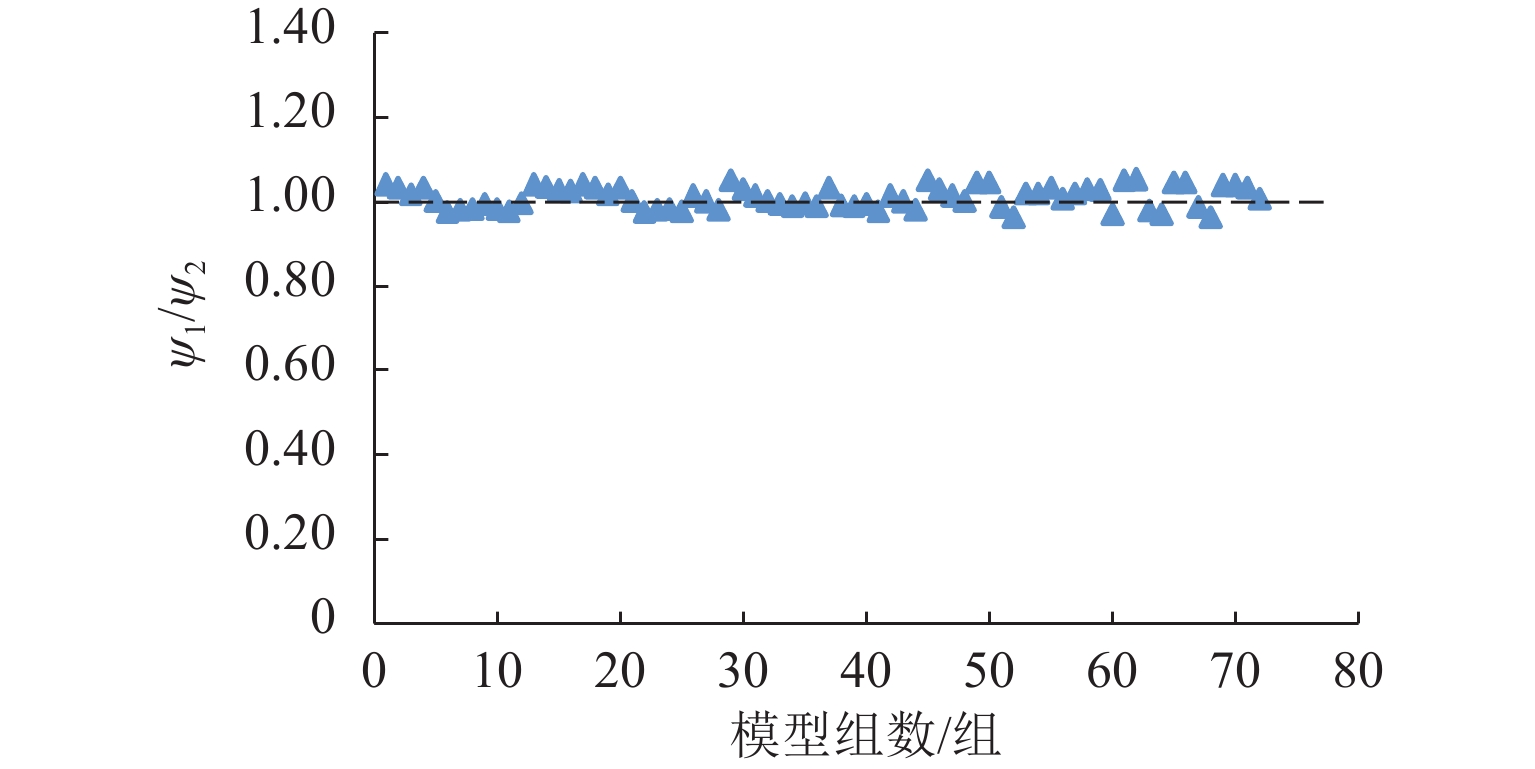
为解决输电角钢塔大荷载作用下的地螺连接问题,提出一种内嵌式十二地螺节点结构. 基于刚度差异演化的塑性屈服线理论,考虑地螺位置、地螺与塔脚板刚度差异的影响,推导出上拔荷载作用下内嵌地螺与外地螺内力不均匀计算的理论公式;然后,结合24组精细化数值分析,研究不同地螺直径、地螺间距和塔脚板厚度对内、外地螺上拔内力差异的影响,并对其刚度差异修正系数进行标定;最后,通过72组数值试验验证理论公式的合理性. 研究结论表明:内嵌式十二地螺结构较传统八地螺结构可以提高节点上拔承载力40%~50%;当内地螺布置在外地螺正交线上时,内、外地螺在上拔荷载作用下的内力不均匀分布系数约为1.1,且不随地螺直径和塔脚板厚度变化;当内地螺沿着对角线靠近外地螺时,内地螺内力的不均匀分布系数减小,但当塔脚板厚度增大时,不均匀分布系数会增大;考虑刚度差异演化的塔脚板塑性屈服线理论的计算结果与数值模拟结果相比,理论不均匀分布系数与数值不均匀分布系数比值的均值为1.01,变异系数为0.03.
Abstract:In order to solve the problem of ground screw connection under large load of transmission angle steel tower, an embedded twelve ground screw joint structure was proposed. Based on the plastic yield line theory of stiffness difference evolution, the influence of the position of ground screws and the stiffness difference between ground screws and tower foot plates was considered, and the theoretical formula of uneven internal force between embedded ground screws and external ground screws under uplift load was derived. Then, combined with 24 sets of refined numerical analysis, the influence of different ground screw diameters, ground screw spacings, and tower foot plate thicknesses on the differences in uplift forces of internal and external ground screws was studied, and the correction coefficient of stiffness difference was calibrated. Finally, the theoretical formula was verified by 72 sets of numerical experiments. The research results show that the embedded twelve ground screw structure can improve the uplift bearing capacity of the joint by 40%–50% compared with the traditional eight ground screw structure; when the internal ground screw is arranged on the orthogonal line of the external ground screw, the uneven distribution coefficient of internal force of the internal and external ground screws under the uplift load is about 1.1, and it does not change with the diameter of the ground screw and the thickness of the tower foot plate. When the internal screw is close to the external screw along the diagonal, the uneven distribution coefficient of the internal force of the internal screw will decrease, but when the thickness of the tower foot plate increases, the uneven distribution coefficient will increase. The calculation results based on the plastic yield line theory of stiffness difference evolution of the tower foot plate are compared with the numerical simulation results. The average value of the ratio of the theoretical uneven distribution coefficient to the numerical uneven distribution coefficient is 1.01, and the coefficient of variation is 0.03.
-
泡沫铝作为多孔材料的一种,由于其低密度、高孔隙率且能够在稳定的应力水平下发生较大的塑性变形来耗散能量等优点[1-3],现已成为一种理想的缓冲吸能材料被广泛应用. 泡沫铝材料在工程应用时既能满足轻量化的设计需求,又能显著提高结构的抗冲击性能,目前更多地应用于汽车、航空等领域,提高载运工具的运行安全.
由于泡沫铝材料受压时表现出良好的吸能特性,引起研究人员对其压缩性能的关注. 目前已经有很多国内外研究学者对影响泡沫铝力学性能的基本因素展开了研究,包括基体材料属性、相对密度和应变率等:郭亚周等[4]分析了泡沫铝在中低应变率、不同相对密度和不同泡沫铝基体材料下的力学承载性能变化,研究结果表明中低应变率下泡沫铝不具备应变率效应,此外,相对密度较小的泡沫铝具有更高的吸能效率;李忠献等[5]采用改进的INSTRON高速动力加载系统对闭孔泡沫铝的动态压缩性能展开了研究,结果表明泡沫铝应力随着应变率的提高而增大,材料的能量吸收特性也随之提升;Wang等[6]对闭孔泡沫铝进行了不同速度下的直接冲击式霍普金森压杆(DHPB)试验,结果表明当冲击速度超过临界值时平台应力动态增强,并将这种增强归因于惯性效应. 此外还有部分学者[7-9]对于温度对泡沫铝压缩性能的影响展开了研究,李雪艳等[7]通过进行不同温度下的泡沫铝压缩试验研究,表明闭孔泡沫铝具有温度软化效应,其压溃强度随温度升高而降低,且与温度变化成线性关系. 在泡沫铝的工程应用方面:郭亚周等[10]通过LS-DYNA开展了机翼前缘未填充和局部填充泡沫铝材料抗鸟撞的分析,结果表明局部填充泡沫铝的机翼前缘能够比空机翼前缘在相同撞击工况下吸收更多的能量;翟希梅等[11]将泡沫铝填充至6082-T6铝合金圆管中制备了耗能复合构件,通过轴压试验证明填充泡沫铝能够有效改善构件在轴压荷载下的变形能力,避免其发生不规则变形破坏,并提高构件耗能能力.
关于泡沫铝的有限元表征模型,国内外不少学者都开展了一定研究,因为泡沫铝内部孔隙杂乱无章分布且不均匀,建模难度较大且内部孔隙结构难以重建. 泡沫铝三维几何模型通常采用连续的实体模型[12-13]或者带有随机孔隙分布的实际结构模型[14-15]:前者通过将泡沫铝压缩力学本构模型赋予实体模型以表征其力学响应,该方法建模简单且计算求解速度快,但无法模拟内部孔隙的准确变形过程,适用于泡沫铝充当吸能结构与其他部件的耦合仿真;后者建模时带有随机分布的孔隙,赋予基体材料力学属性并以此来反映泡沫铝的真实力学属性,较为常用的主要有Kelvin模型和Voronoi模型,此种建模方法难度较大,且计算时间较长,但是可以看出准确的孔隙变形特征.
目前建立的泡沫铝等效有限元模型的研究中尚未较好地考虑应变率效应的问题,本文通过试验和仿真结合的方式建立了能够反映应变率效应的两种密度泡沫铝的等效模型,并将其应用于直升机驾驶舱低空抗坠毁分析当中.
1. 泡沫铝压缩试验
1.1 试验介绍
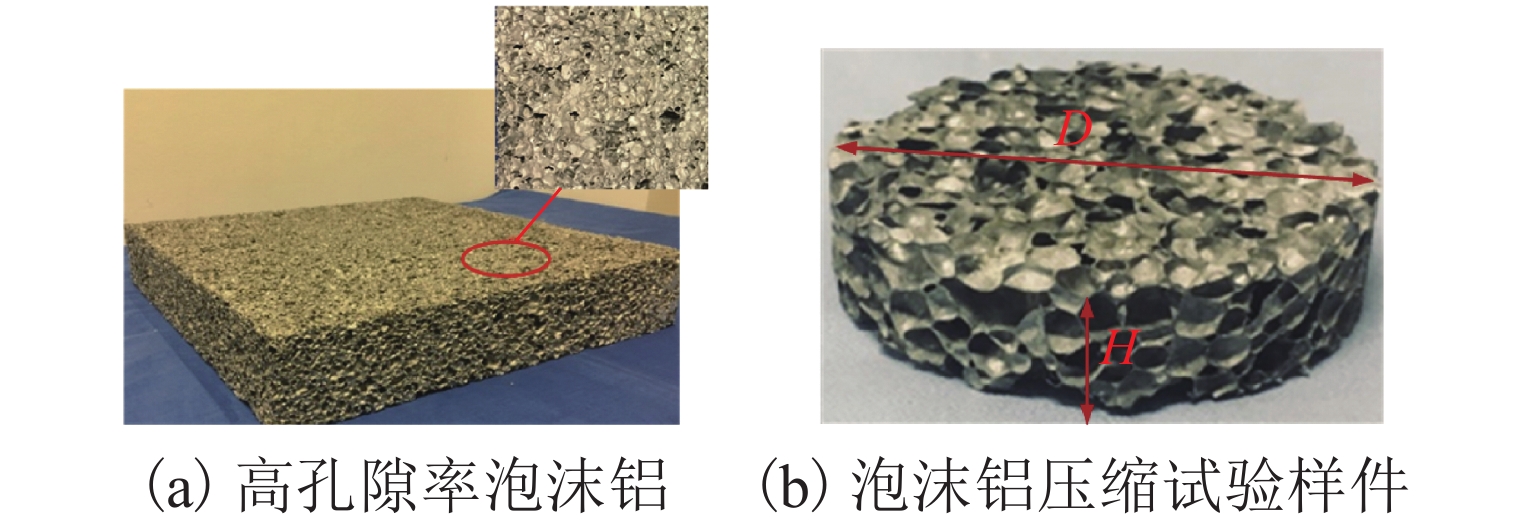
本文中所用试验试样为两种不同孔隙率的闭孔泡沫铝,制备基体材料均为纯铝. 其中高孔隙率泡沫铝的孔径尺寸较大且分布不均,大部分为2~5 mm,相对密度
ρ∗ 较小,仅为0.080;低孔隙率泡沫铝孔径尺寸相对较小且均匀,大部分为2~3 mm,相对密度ρ∗ 较大,为0.126. 为区分上述两种泡沫铝材料,称前者为低密度泡沫铝,称后者为高密度泡沫铝,此处的高低仅表示相对关系.试验试件设计为圆柱形,统一采用线切割进行加工. 考虑到低密度泡沫铝的孔隙直径较大,部分可达5 mm以上. 根据试件设计规则,所有试样在每个方向上都至少有7个胞元[16],以避免压缩过程中可能产生的边界效应和尺寸效应,最终试件直径确定为60 mm,高度为15 mm,其中高孔隙率泡沫铝试件如图1所示.
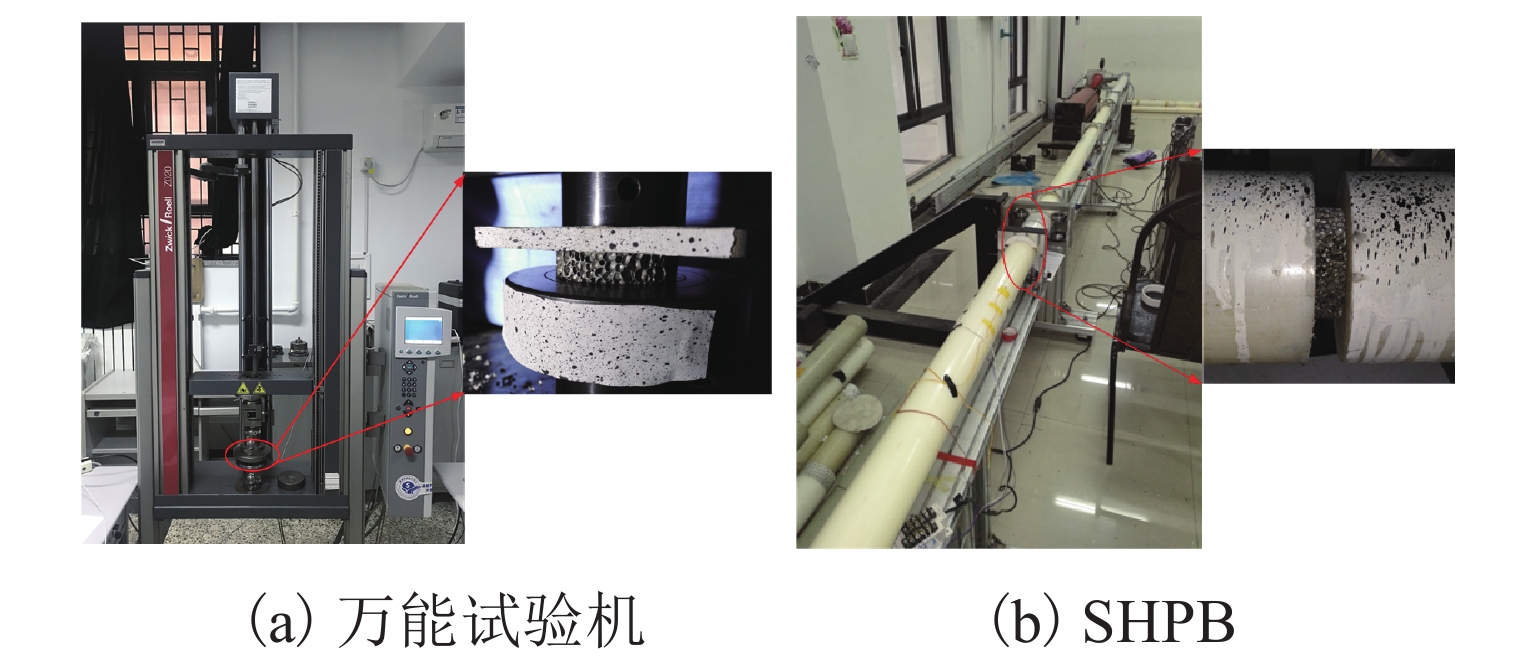
本文对两种不同密度的泡沫铝分别进行了0.001 /s、500 /s、1000 /s不同应变率下的压缩试验. 其中准静态试验在Zwick/Roell Z020型万能试验机上进行,试验装置如图2(a)所示. 试验过程中通过力传感器获取力-时间曲线,通过在试验机压头喷射散斑使用非接触测量(DIC)的方式来获取压头位移,进而获取力-位移曲线. 并根据应力
σ=F/A 、应变ε=d/H 换算得到应力-应变曲线,其中:F为压力;A为试件横截面积;d为压头位移;H为试件高度.通过分离式霍普金森压杆(SHPB)进行中高速试验,试验装置如图2(b)所示,包括高压充气系统、装置控制系统、高速摄像及灯光系统、应变采集仪、试件固定等部分. 试验之前将试件胶粘固定于输出杆端,根据试验应变率估计气压. 入射杆直径为100 mm,弹性模量为3513 MPa,应力波经入射杆传播后在入射杆端发生反射和透射,通过应变片记录入射、透射和反射的信号得到试件两个端面上应力和应变率的相关信息. 采用经典二波法进行试验数据处理. 整个试验持续约2~3 ms,通过高速摄像机采集试验过程,采集的频率约为20000~40000帧/s.
1.2 试验结果
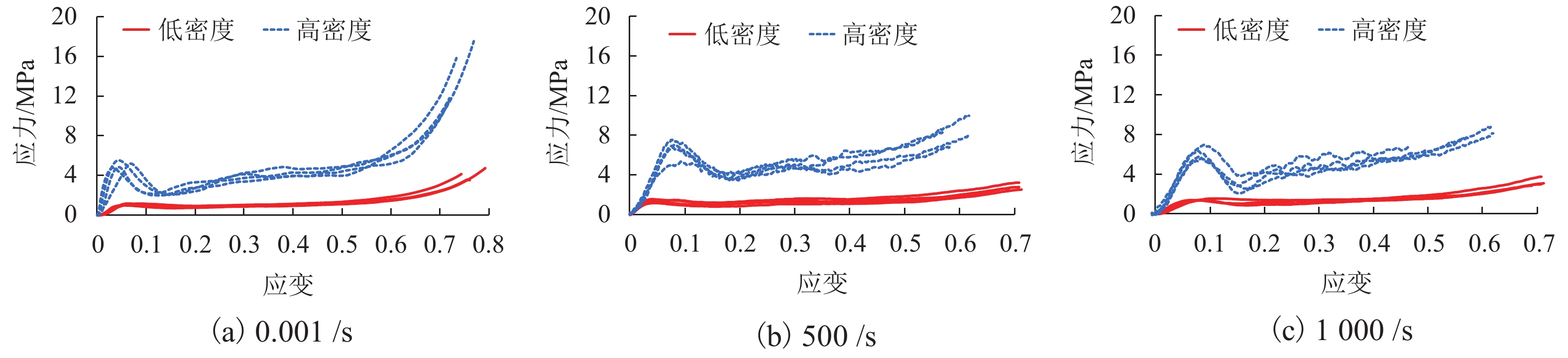
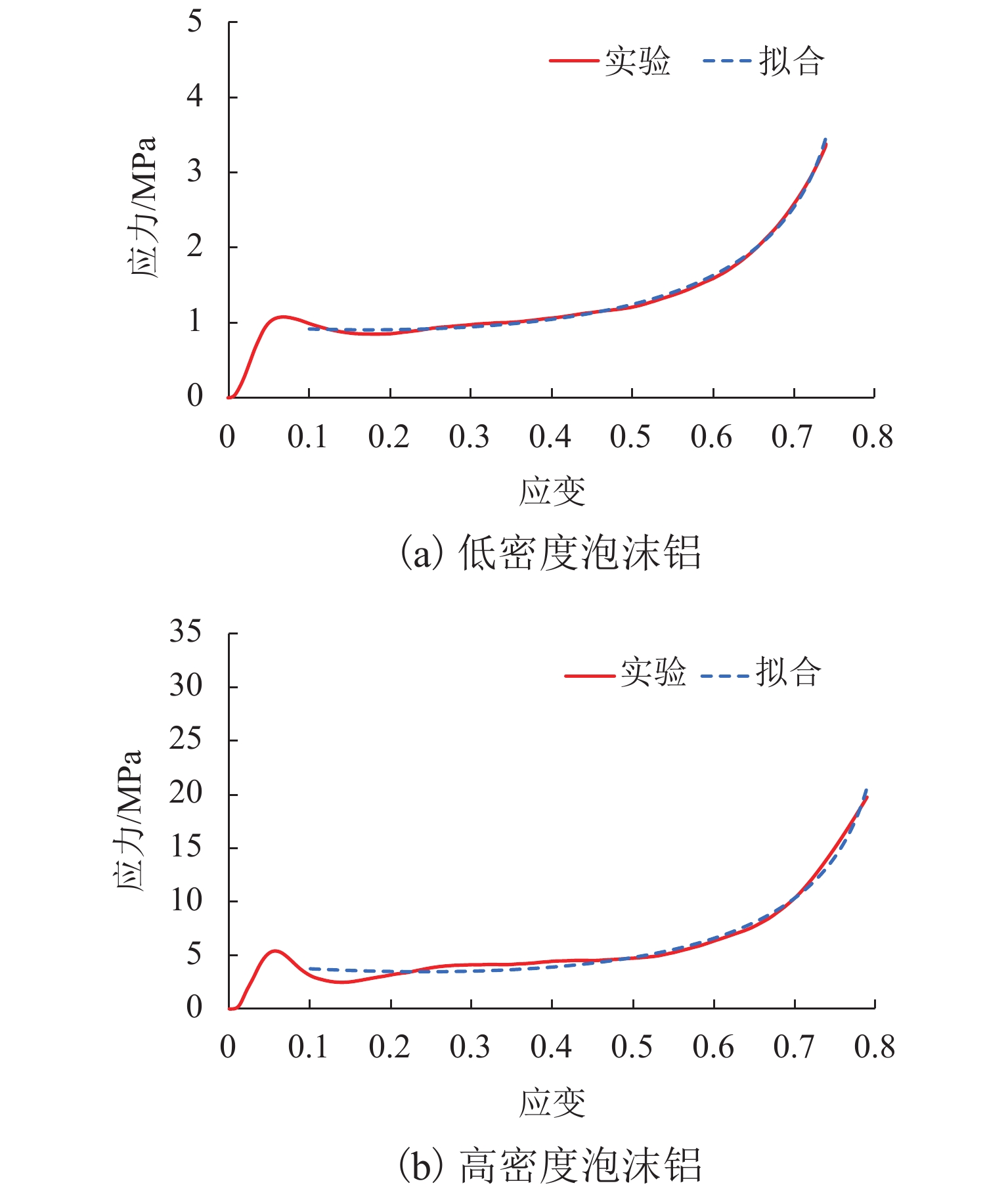
为保证试验结果的可靠性,每个工况都进行4次重复试验取平均值. 两种密度泡沫铝不同工况下的应力-应变曲线及压缩过程如图3、4所示. 由图3可以看出:泡沫铝压缩应力-应变曲线均表现出明显的三段特征,即线弹性、塑性平台段和密实段.
由图4可知:1) 低密度泡沫铝试样孔壁更薄,实际加工过程中必然存在孔壁缺失、孔壁连接部位较弱等缺陷,在压缩过程中首先被压溃,压溃区域周围的孔壁应力升高并重新分布,变形带逐渐延伸,表现为“V”形剪切破坏,该过程重复进行,变形带基本上被压实,该过程应力快速升高,压缩进入密实化阶段. 由于整个过程中应力传递较为均匀,压缩的应力应变曲线比较平滑. 2) 高密度泡沫铝压缩过程前期基本可以保持完整,但后期压缩变形较大时边界发生碎裂,有较多的基体碎屑散落在试样周围,该现象在高速工况下更为显著. 这是因为高密度泡沫铝内部胞壁的缺陷很少,只能通过孔壁的断裂而非塑性变形来吸能,导致压缩过程中应力传递不均匀,波动较大. 此外随着应变率的增加,平台段应力值提升更快,且平台段的波动更加明显. 这是由于压溃过程中,周围孔壁应力来不及发生重分布,坍塌带迅速扩展导致的. 随着应变率的提升,应力波动现象愈发明显.
对于泡沫铝等耗能缓冲构件,常采用比吸能和平台应力作为评价其承载吸能能力的重要指标. 其中比吸能(SEA)代表结构单位质量或单位体积下的吸能能力,比吸能越大,泡沫铝的承载吸能能力越好. 质量比吸能及体积比吸能的计算方法如式(1).
Sm=EAm,SV=EAV, (1) 式中:m和V分别为泡沫铝的质量和体积;EA为压缩开始至密实化应变区间内泡沫铝的总吸能量,本文取应力-应变曲线中对应1.3倍平台应力[16]处的应变值作为密实化应变.
平台应力(
σpl )作为评估泡沫铝压溃过程中吸收能量的关键参数,如式(2).σpl=1ε2−ε1∫ε2ε1σ(ε)dε, (2) 式中:
ε1 和ε2 分别为泡沫铝轴向承载时平台段的起始应变和密实化应变.不同压缩应变率工况下泡沫铝的压缩结果如表1所示,各指标均为4次压缩试验的均值.
表 1 不同密度泡沫铝压缩试验结果Table 1. Test results of aluminum foams with different densities试件 应变
率/(s−1)平台应
力
/MPa密实化
应变质量比吸能/
(kJ·kg−1)低密度
试件0.001 0.95 0.57 1.88 500 1.31 0.53 2.75 1000 1.37 0.50 2.89 高密度
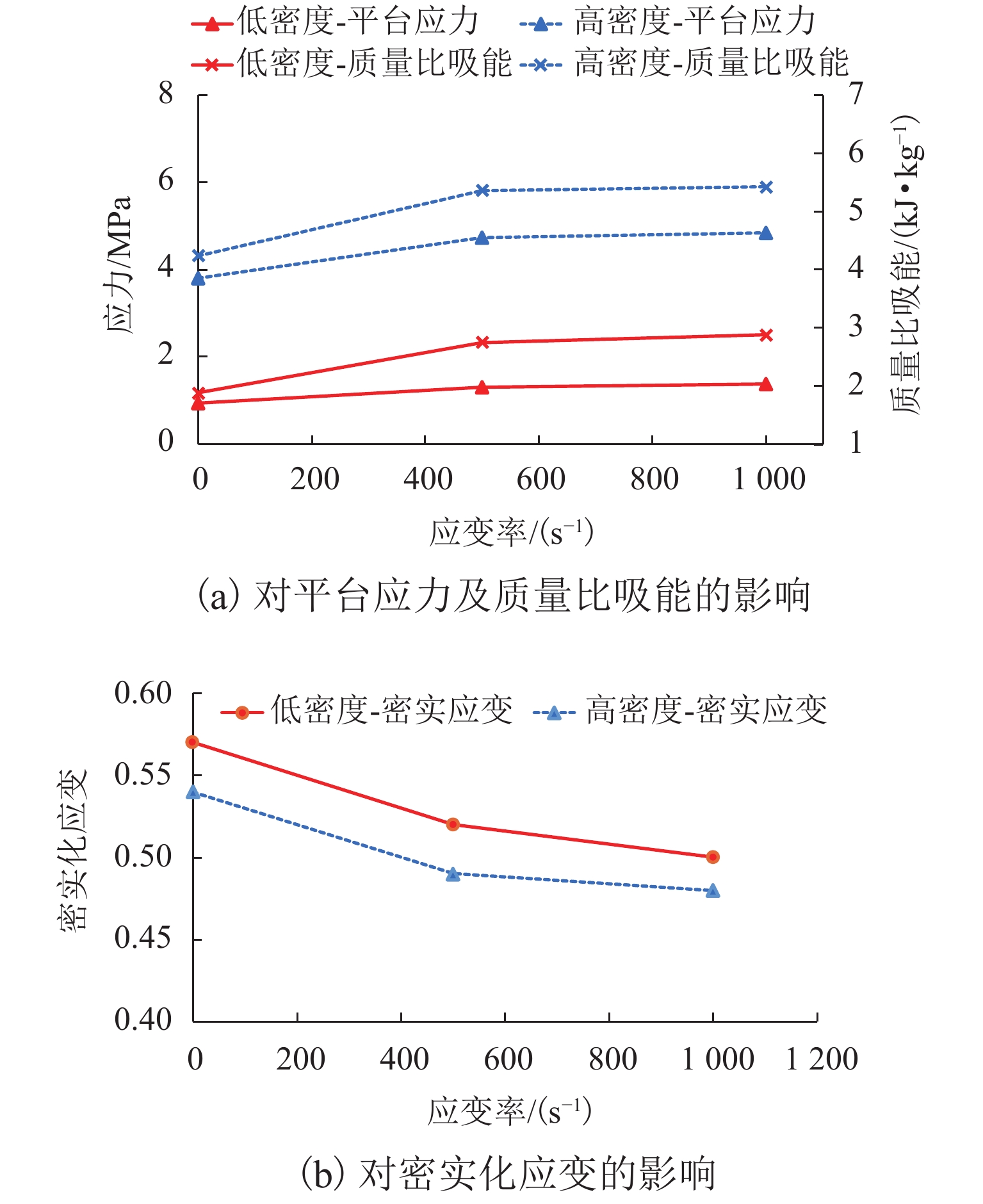
试件0.001 3.80 0.55 4.24 500 4.73 0.49 5.36 1000 4.85 0.48 5.42 1.3 相对密度及应变率对承载能力的影响规律
泡沫铝材料的初始相对密度以及应变率对其力学性能承载指标影响显著,其影响规律如图5所示. 由图5可知:1) 无论哪种相对密度的泡沫铝,平台应力以及质量比吸能都随应变率的增加而增加,表现出明显的应变率敏感性. 随着相对密度的增大,两个指标的提升现象变得不明显. 2) 无论哪种相对密度的泡沫铝,其压缩过程中的密实化应变均随应变率的增加而呈现出下降趋势. 这是因为在泡沫铝的压溃过程中,孔隙壁的屈曲和坍塌是主要的吸能方式,在低应变率下,孔隙壁有足够的时间来进行旋转变形使得遗留孔隙最小,然而在高应变率下,在下一阶段孔隙壁变形之前,前一阶段孔隙壁没有足够的时间变形至最终模式,所以导致致密模式发生改变,引起了致密化应变的减小,这与文献[17]中的结论一致.
2. 考虑应变率效应的泡沫铝压缩等效模型建立
2.1 等效模型建立
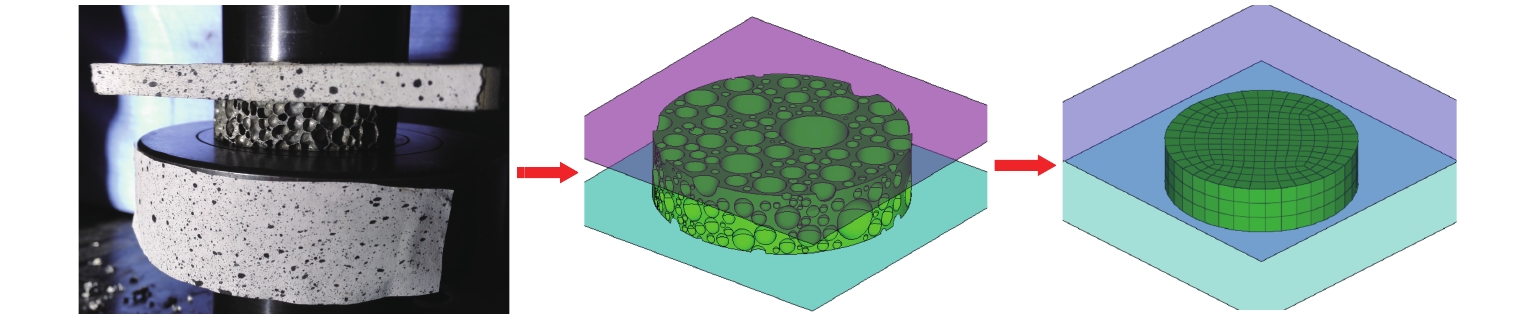
为了评估泡沫铝在直升机驾驶舱低空坠落工况下的承载吸能效果,需要建立可用于驾驶舱垂直跌落仿真的泡沫铝模型. 考虑到泡沫铝内部由无规律分布的孔隙组成,如果建立其原样准确模型用于驾驶舱跌落的仿真,泡沫铝较小的网格尺寸与驾驶舱模型中的大网格尺寸的时间步长不匹配,导致耦合仿真计算时间难以接受. 因此,本文基于HyperMesh和LS-DYNA平台建立了可表征应变率效应的不同密度的泡沫铝有限元等效模型. 该等效模型在进行耦合仿真时可以大幅减少计算时间,并且可以真实反映出泡沫铝压缩试验中所表现出的力学特性以及应变率效应以保证仿真结果的准确性,能在实际工程中进行应用. 泡沫铝等效有限元模型如图6所示,该模型分为3部分:泡沫铝试件、顶部加载刚性板以及底部约束刚性板.
为了验证泡沫铝等效模型的有效性,利用上述建模方式建立了与试验尺寸信息一致的有限元模型. 泡沫铝模型为
ϕ60×15 mm的圆柱体. 泡沫铝模型置于上下两块刚性平板中间. 下刚性板施加全固定约束,上刚性板只有压缩方向1个移动自由度. 对上刚性板施加强制位移使其冲击泡沫铝模型以模拟压缩工况.由于试验过程中泡沫铝试件发生横向膨胀变形后与加载刚性板之间的接触界面节点发生了改变,因此选用自动面-面接触来模拟泡沫铝与上下刚性板的接触,摩擦系数设为0.3. 对泡沫铝施加CONTACT_INTERIOR接触防止出现负体积. 上下刚性板以及泡沫铝模型均采用八节点六面体单元进行网格划分,积分方式为0号,沙漏类型为2号[18].
2.2 材料模型
采用刚性材料(MAT_20)模拟两端刚性板. 泡沫铝采用MAT_MODIFIED_HONEYCOMB本构进行模拟,对于所有的法向应力和切向应力,可以分别定义其非线性弹塑性行为. 通过在3个方向上指定相同的材料属性可以实现各向同性.
该材料模型遵循式(3)所示的应力强化规律[19].
σ=σp+γˆεεD+αln[11−(ˆε/εD)β], (3) 式中:
σ 和ˆε 分别为结构当前的应力和应变;σp 为初始平台应力;等式右边第二项为线性应变硬化项,其中线性应变硬化系数γ 为应力-应变曲线的斜率,εD 为密实化应变,εD=−ln(ρf/ρf0) ,ρf 为泡沫铝的密度,ρf0 为泡沫铝基体材料的密度;等式右边最后一项表示非线性应变硬化,由系数α (比例系数)和β (形状系数)表示.使用Origin自带的用户自定义拟合函数的非线性拟合工具对两种不同密度泡沫铝准静态压缩工况下的应力-应变曲线进行本构方程式(3)的拟合,具体的拟合方法是将初始平台应力作为定值,其余4个参数作为变量,采用Levenberg-Marquardt优化迭代算法进行迭代求得最优的拟合参数. 两种密度泡沫铝的本构拟合参数如表2所示,其中:
EAAU、EBBU、ECCU 分别为3个正交方向下的弹性模量;GAAU、GBBU、GCCU 为3个方向下的剪切模量. 结果如图7所示.此外该本构的杨氏模量和剪切模量从未开始压缩状态到压实状态随体积应变变化而线性变化,初始状态值需要用户给定. 两种不同密度泡沫铝的初始弹性模量由加载卸载试验来确定,试验结果表明低密度泡沫初始弹性模量为300 MPa,高密度泡沫初始弹性模量为800 MPa. 将两种密度泡沫铝准静态压缩下的应力-应变曲线作为基准应变率下的应力-应变曲线,两种密度泡沫铝的材料参数如表2所示.
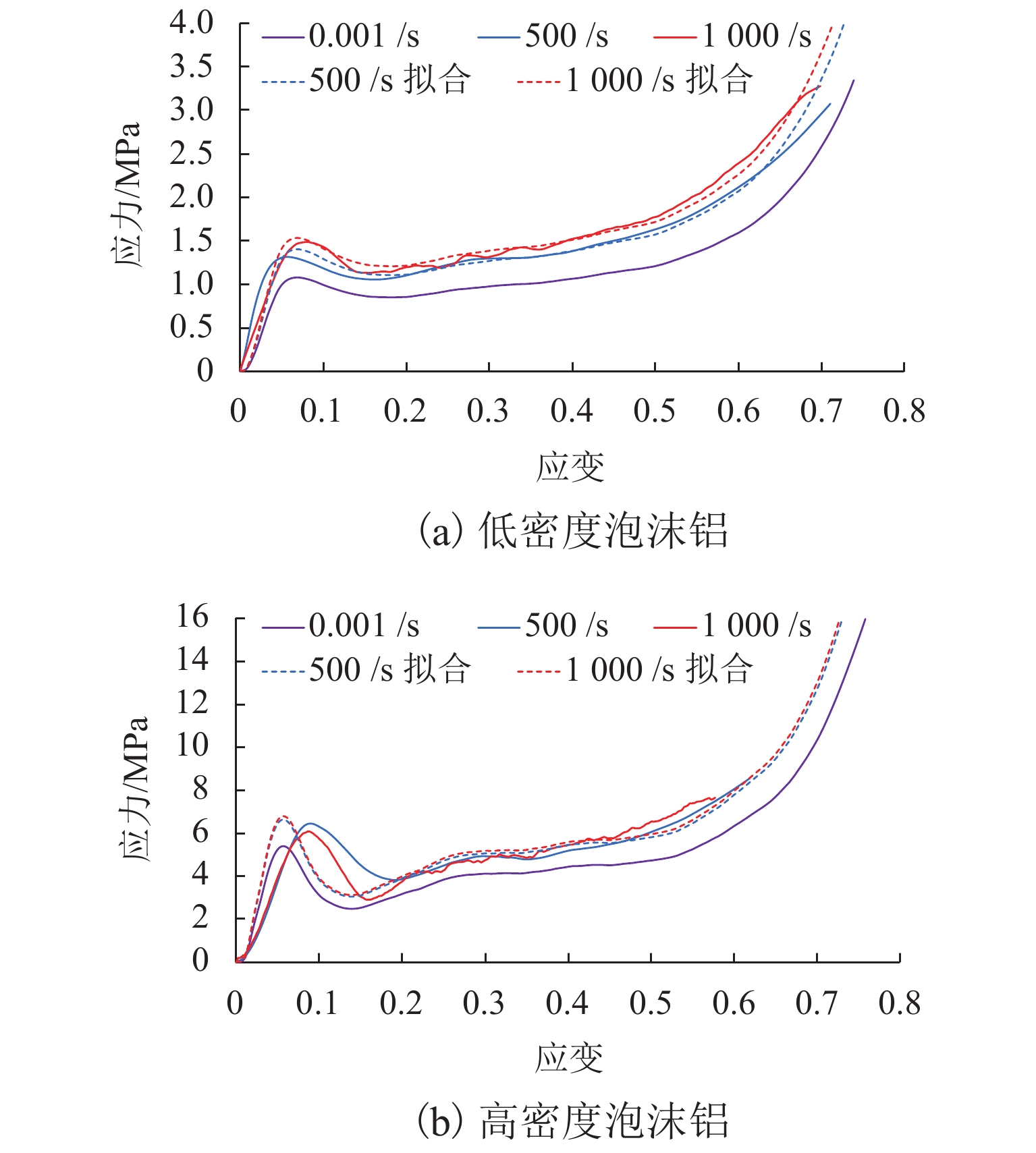
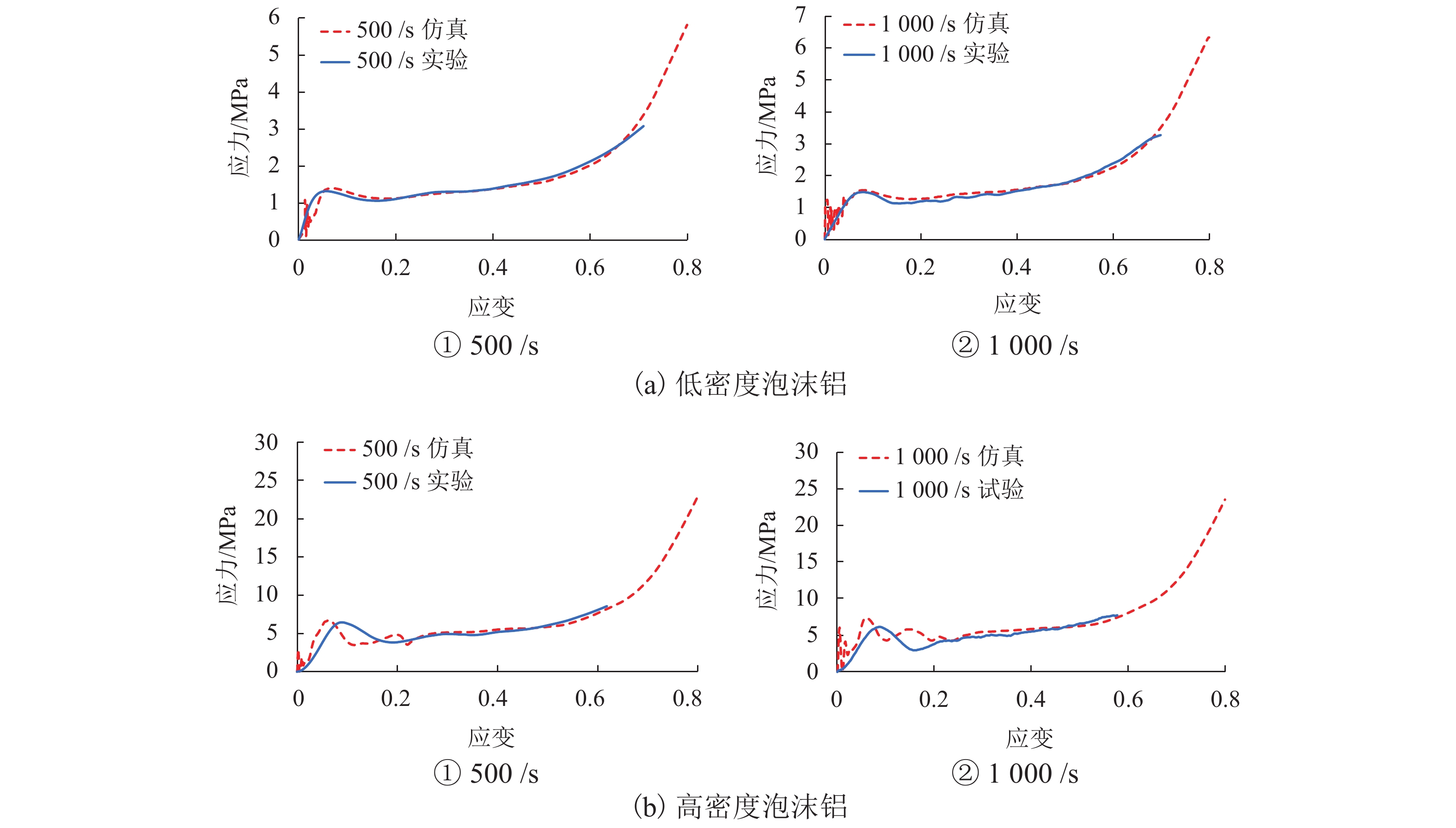
该本构对于应变率效应的表征是通过首先确定不同应变率相较于基准应变率的缩放系数,然后对基准应变率下的应力-应变曲线进行缩放得到不同应变率下的力学响应曲线. 通过试验结果,将两种密度泡沫铝基准应变率(0.001 /s)下得到的压缩应力-应变曲线分别进行缩放以拟合相同密度泡沫铝在500 /s以及1000 /s应变率下的应力-应变曲线. 因为平台段作为泡沫铝压缩承载的关键阶段,因此,缩放标准要保证缩放曲线与原曲线的平台段基本一致,缩放拟合结果如图8所示,得到了两种不同密度泡沫铝在0.001 /s、500 /s、1000 /s应变率下的缩放系数.
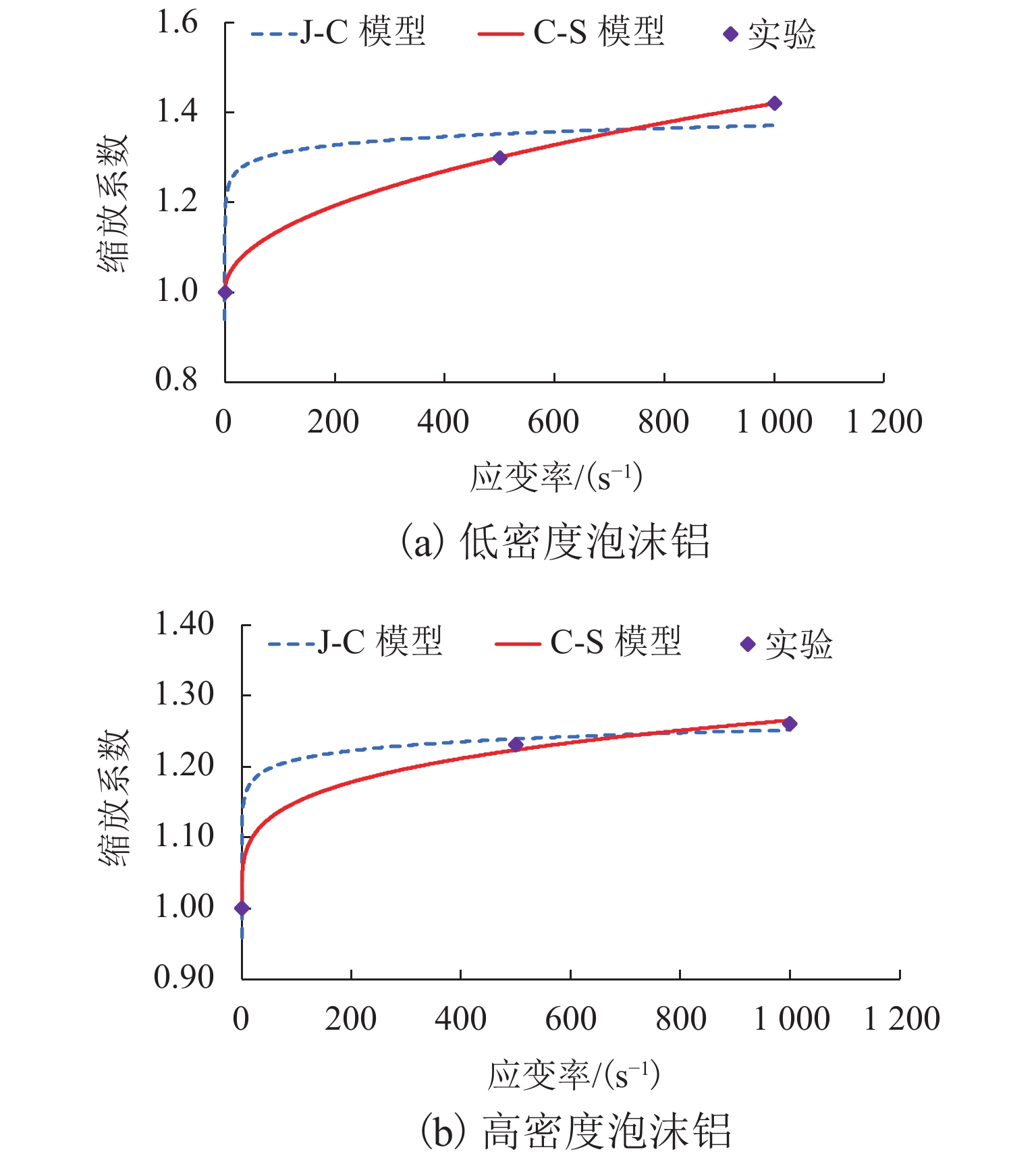
为了表征泡沫铝的应变率效应,需要在本构方程中采用准确的应变率项. 在确定了两种密度泡沫铝基准应变率(0.001 /s)、500 /s、1000 /s应变率下的数据点之后,使用Johnson-Cook(J-C)以及Cowper-Symonds(C-S)两种常用应变率模型进行标定. 其中,J-C模型应变率项采用对数函数表征应变率效应,而C-S模型采用幂函数来表征应变率效应,两者的标定结果如图9所示.
由图9可以看出:1) J-C模型对高密度泡沫铝的应变率效应描述较好,但对于低密度泡沫铝应变率的描述则与试验结果有较大的偏差. 这是由于泡沫铝不同应变率下的缩放系数在对数坐标下并非线性,而J-C模型应变率项为线性函数导致的. 2) 反观C-S模型的标定结果与试验结果吻合较好,相关系数达到0.99,故采用C-S模型作为最终泡沫铝应变率效应的表征函数,如式(4),标定系数如表3所示. 将该标定结果曲线作为泡沫铝等效模型应变率项的输入以准确表征泡沫铝的应变率效应.
表 3 应变率模型标定系数Table 3. Calibrated coefficients of the strain-rate model泡沫铝 b e 相关系数(R2) 低密度 5.969 × 106 0.485 0.999 高密度 2.112 × 108 0.248 0.992 {f}_{{\rm{rate}}}=1 + {\left(\dfrac{\dot \bar \varepsilon _{\rm{p}}^*}{b}\right)}^{e}, (6) 式中:
frate 为不同应变率下的缩放系数;{\dot \bar \varepsilon _{\rm{p}}^*} 为等效塑性应变率; e和b为 需要拟合的材料参数.2.3 等效模型结果验证
由于0.001 /s应变率下的仿真计算时间过长,因此本节只进行500 /s和1000 /s中高应变率下的仿真结果验证. 两种密度所对应的500 /s和1000 /s应变率下的仿真和试验应力-应变曲线的结果对比如图10所示. 由图10可以看到:除了仿真结果因为初始接触速度大而有所振荡之外,吸能的关键区域平台段曲线结果吻合较好,可以说明该模型具有较高的精度,可以代替真实泡沫铝模型进行仿真从而节约大量的计算时间.
3. 泡沫铝在直升机驾驶舱抗坠毁设计中的应用
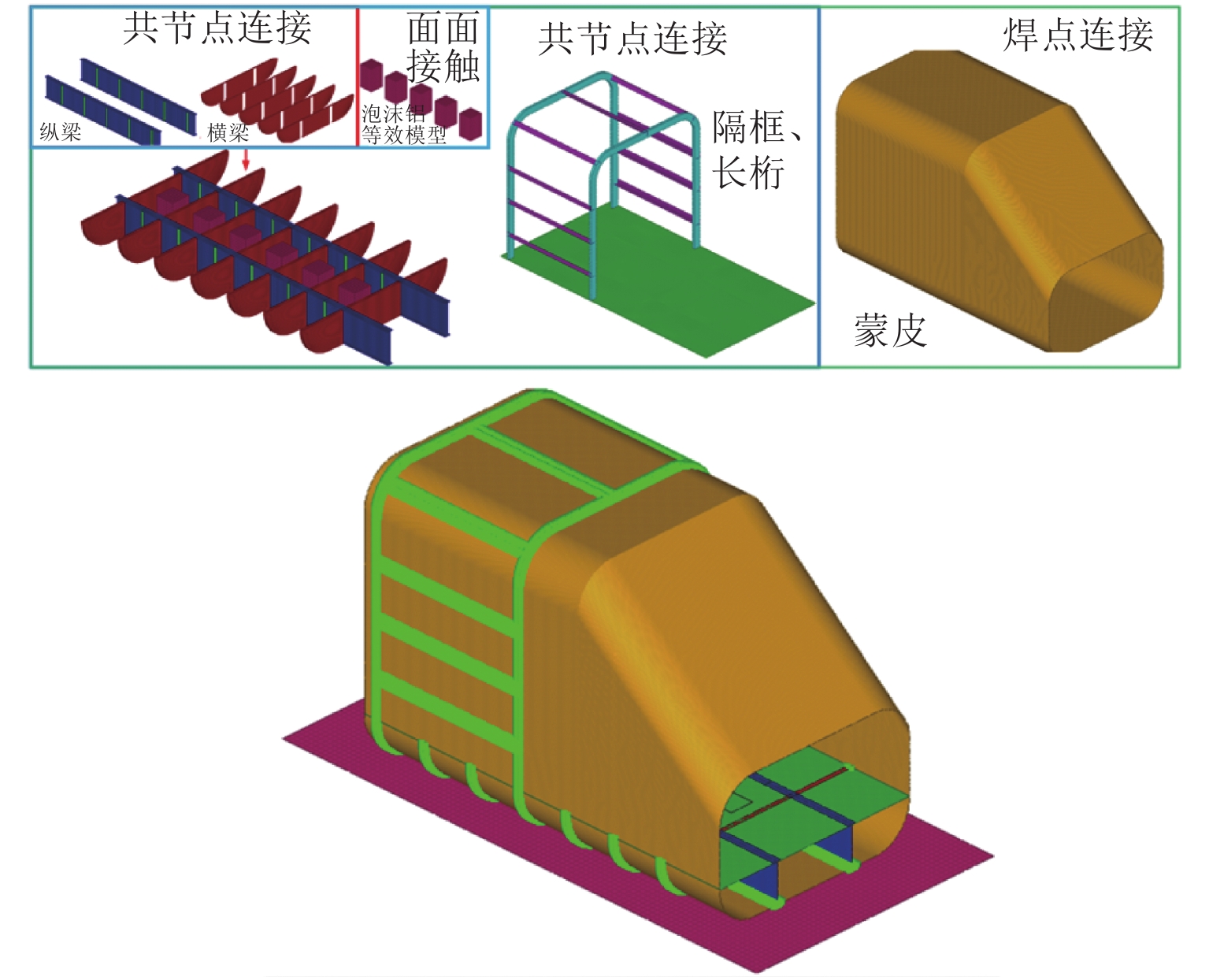
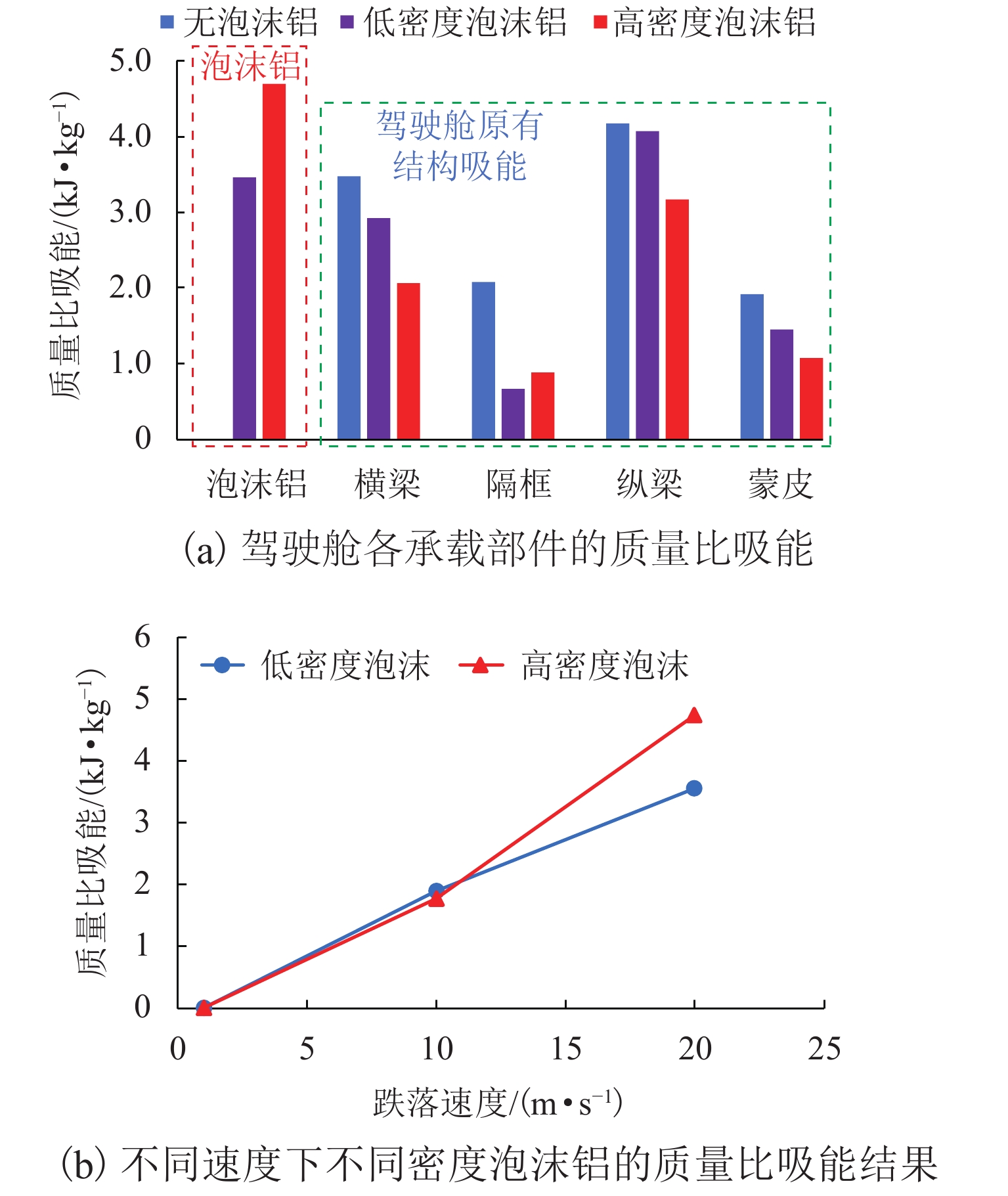
基于建立的可反映应变率效应的不同密度泡沫铝的等效模型,以某型号直升机结构为建模依据,建立了简化后的直升机驾驶舱低空垂向跌落的有限元模型. 通过进行驾驶舱有限元模型和泡沫铝等效模型的耦合跌落仿真,研究泡沫铝密度对于驾驶舱垂向跌落的影响. 该模型由蒙皮、长桁、隔框、底板、横梁、纵梁、支撑板件、泡沫铝等效模型以及底部刚性墙组成,有限元模型如图11所示. 其中泡沫铝的尺寸是根据横纵梁交错位置的空间大小确定的,最终尺寸为180 mm × 176 mm × 300 mm,其安装位置是驾驶舱底部的横纵梁交接空隙处.
该有限元模型各部分的连接关系为:长桁、隔框、底板、横纵梁等内部结构采用共节点接触;长桁、隔框、横纵梁等均与外部蒙皮采用MAT-100 Spotweld焊点连接来模拟铆接;内部泡沫铝等效模型与蒙皮和底板之间采用自动面面接触,驾驶舱模型整体采用自接触,并且与刚性墙之间采用自动面面接触. 对底部刚性墙进行完全固定约束,对驾驶舱模型施加大小为1、10、20 m/s的初始跌落速度以模拟驾驶舱模型低空跌落的工况. 3种跌落速度反映在泡沫铝模型上,对应的单元应变率分别为1 /s、10 /s、30 /s左右. 该模型中,驾驶舱结构均为铝合金材料,采用MAT 24本构进行模拟,统一采用四节点壳单元进行网格划分,泡沫铝等效模型建模与第2节所述一致.
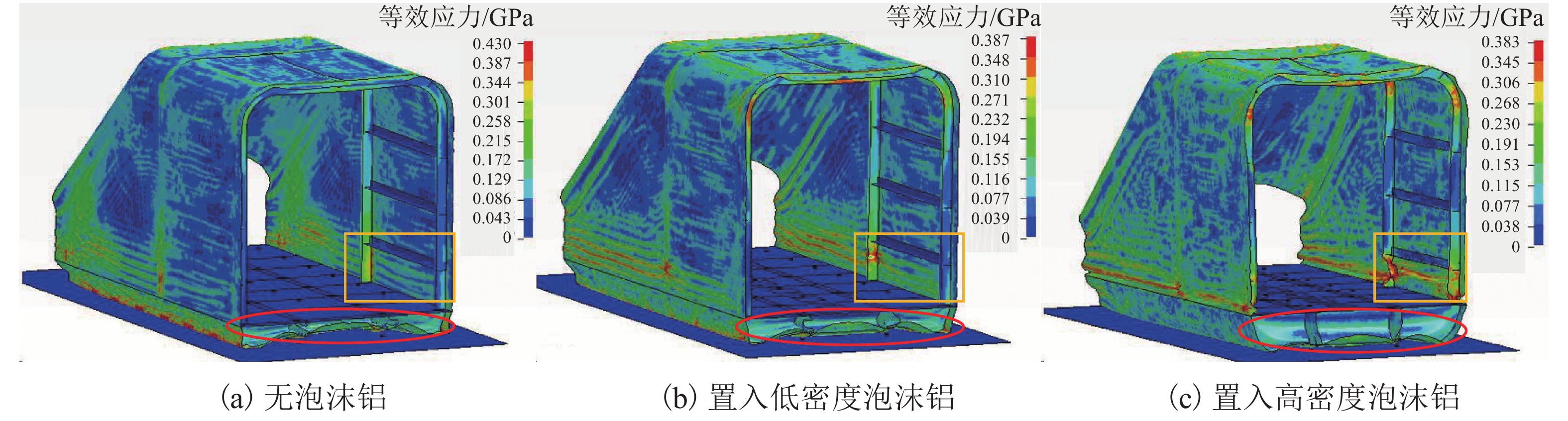
无泡沫铝、置入低、高密度泡沫铝模型的低空跌落仿真变形不同. 以20 m/s的初始跌落速度为例,相同时刻下3种模型的仿真过程如图12所示. 由图12可知:无泡沫铝驾驶舱下部结构的蒙皮、横纵梁首先受到冲击,出现屈曲塑性变形,随着撞击过程继续,撞击能量继续向上传递,底部基本压溃,上部的隔框、长桁以及蒙皮开始发生塑性折弯变形来吸能;填充泡沫铝的驾驶舱模型,横纵梁发生屈曲塑性变形的同时,填充泡沫铝受压发生塑性压缩变形吸收了很大一部分碰撞动能;相较无泡沫铝的驾驶舱模型,相同时刻下底板下部结构变形减少,如红色椭圆部分所示.
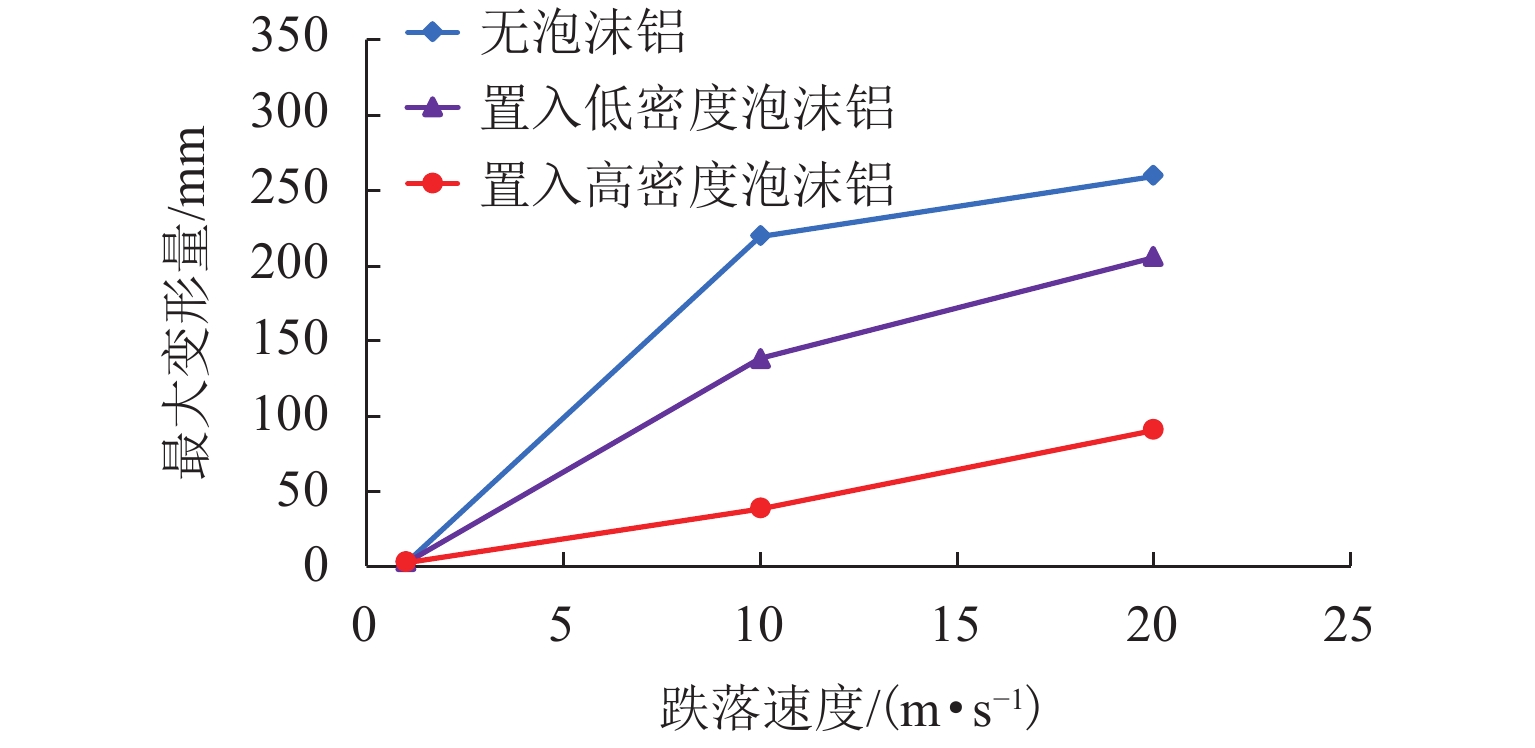
3种不同跌落速度下的最大变形量具体数据如图13所示,泡沫铝起到了明显的缓冲效果,底舱最大变形量得到有效减少. 此外随着跌落速度的增加,碰撞工况更加剧烈,底舱的变形量逐渐增大. 由于泡沫铝的填充,驾驶舱底部的承载能力提高,上部隔框等承载部件更早地进入塑性变形,变形加大,如图12黄色框线部分所示. 由于高密度泡沫铝承载能力高于低密度泡沫铝,因此相同时刻下其底部结构变形更小,但隔框等结构变形更严重.
驾驶舱结构跌落中主要依靠机身结构各部件发生不同程度的塑性变形来吸收碰撞动能,主要吸能形式是底部横纵梁组件的弯曲、内部泡沫铝的塑性压缩变形以及蒙皮、隔框的塑性折弯变形. 3种跌落速度工况下,内部部件的吸能规律基本保持一致,典型工况20 m/s跌落过程中各个主要部件的质量比吸能如图14所示. 可以看出,通过置入泡沫铝结构在压溃过程中吸收大量的碰撞动能,大大缓解了驾驶舱原有结构的承载压力,原有结构吸收能量减少. 这就意味着原有主要承载结构如横纵梁等的设计要求可以降低,承载能力留有一定的冗余,安全性能得到进一步保证. 随着跌落速度的增加,模型输入的初始碰撞动能增加. 跌落过程中,各个承载部件的变形更加严重,吸收能量也有所提高. 以泡沫铝结构为例,不同跌落速度下的泡沫铝质量比吸能如图14(b)所示,可以看出,随着初始跌落速度的增加,不论何种密度的泡沫铝的质量比吸能均随着跌落速度的增加而增加. 此外相对密度更大的泡沫铝在实际承载中体现出更高的能量吸收能力.
4. 结 论
本文首先进行了不同应变率、不同相对密度泡沫铝的压缩试验,研究了不同相对密度的闭孔泡沫铝的力学性能;然后,建立了可放映应变率效应的泡沫铝等效有限元模型并应用于直升机耐坠性设计中,得出了以下结论:
1) 低密度泡沫铝压缩过程更为稳定,高密度泡沫铝压缩过程波动较大,且有碎屑脱落.
2) 泡沫铝压缩时的平台应力和质量比吸能随相对密度及应变率的增加而增大,但密实化应变相反,随相对密度或应变率增加而减小.
3) 基于MAT_MODIFIED_HONEYCOMB本构建立了可反映应变率效应的不同密度泡沫铝的等效有限元模型,经过试验结果验证具有较高的精度.
4) 置入泡沫铝后驾驶舱原有各部件的承载压力和底舱的最大变形量均得到有效的减小,内部乘客的生存空间可以得到有效的保证,高密度泡沫铝相较低密度泡沫铝吸能效果更佳.
-
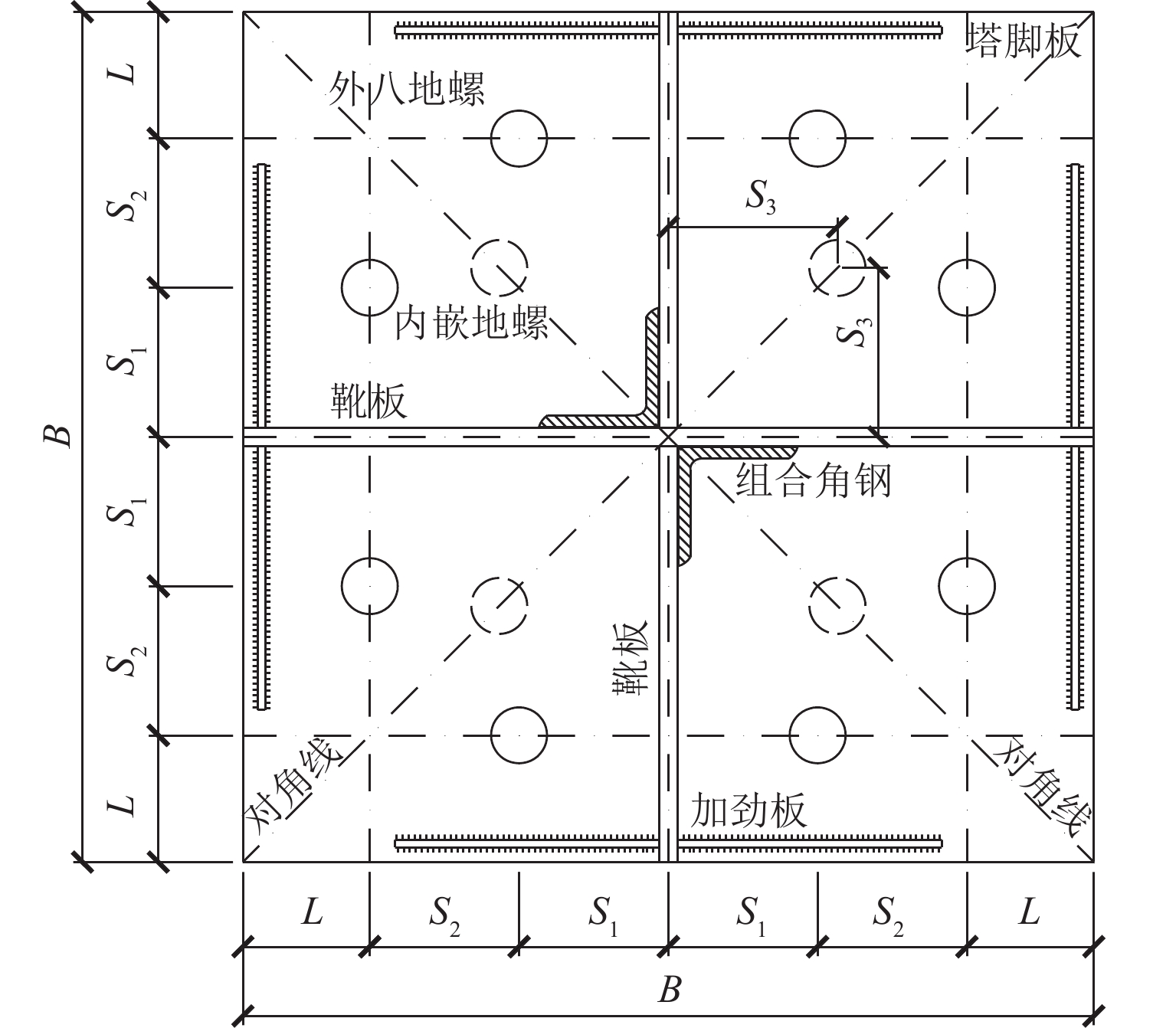
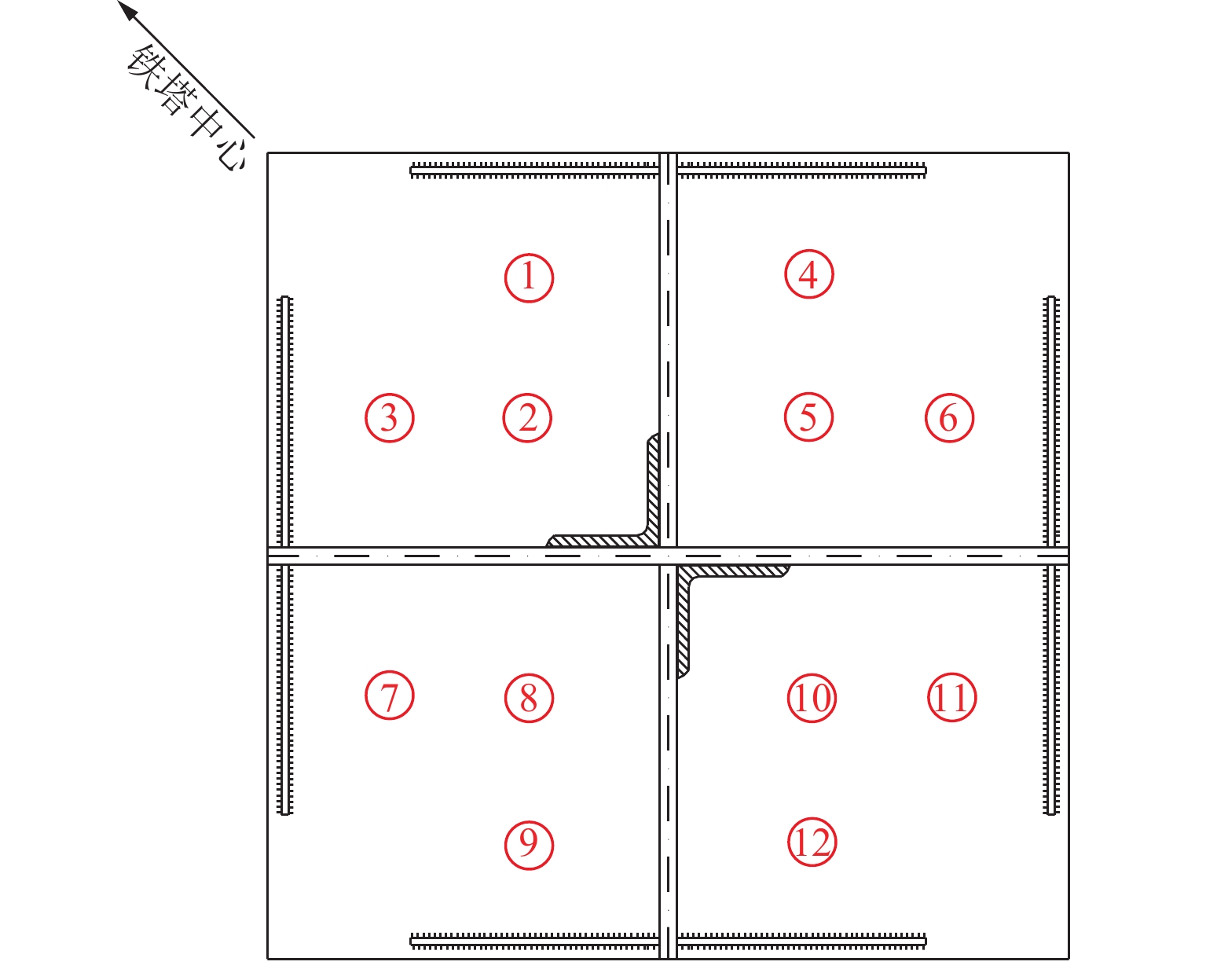
表 1 尺寸参数
Table 1. Dimension parameters
mm 地螺
模型S1 S2 S3 L t 12M64 180 185 180.0,202.5,220.0 150 45,55,65,75 12M72 200 205 200.0,225.0,250.0 170 45,55,65,75 表 2 规格及荷载
Table 2. Specifications and loads
地螺模型 主材规格 地螺承载
力/kN施加荷载/
kN应力比 12M64 2L250 × 28 9954.7 8500 0.85 12M72 2L280 × 35 12871.2 11000 0.85 表 3 12M64地螺内力对比分析(S3 = 180.0 mm)
Table 3. Comparative analysis of internal force of 12M64 ground screw (S3 = 180.0 mm)
地螺
编号t = 45 mm t = 55 mm t = 65 mm t = 75 mm N/kN ψo N/kN ψo N/kN ψo N/kN ψo 1 716 1.08 695 1.11 677 1.10 694 1.06 2 745 755 740 724 3 669 662 674 668 4 741 1.04 759 1.03 741 1.06 735 1.05 5 730 738 753 747 6 668 667 674 683 7 765 1.02 755 1.02 751 1.03 753 1.03 8 726 733 740 740 9 656 678 682 681 10 756 1.14 744 1.13 765 1.17 758 1.15 11 648 641 654 648 12 681 672 649 670 ¯ψ 1.07 1.08 1.09 1.08 表 4 12M64地螺内力对比分析(S3 = 202.5 mm)
Table 4. Comparative analysis of internal force of 12M64 ground screw (S3 = 202.5 mm)
地螺
编号t = 45 mm t = 45 mm t = 65 mm t = 75 mm N/kN ψo N/kN ψo N/kN ψo N/kN ψo 1 727 1.00 720 0.96 694 1.01 684 1.03 2 708 685 698 709 3 694 702 691 686 4 790 0.91 798 0.91 782 0.97 767 1.00 5 677 675 704 722 6 698 694 672 681 7 791 0.87 791 0.90 775 0.92 752 0.99 8 653 674 690 717 9 703 699 720 699 10 678 0.98 697 1.02 709 1.04 717 1.05 11 690 681 695 682 12 690 682 671 684 ¯ψ 0.94 0.95 0.98 1.02 表 5 12M64地螺内力对比分析(S3 = 220.0 mm)
Table 5. Comparative analysis of internal force of 12M64 ground screw (S3 = 220.0 mm)
地螺
编号t = 45 mm t = 55 mm t = 65 mm t = 75 mm N/kN ψo N/kN ψo N/kN ψo N/kN ψo 1 762 0.76 762 0.81 720 0.90 710 0.93 2 583 602 642 654 3 777 731 706 702 4 826 0.72 813 0.80 797 0.85 759 0.93 5 569 617 646 687 6 746 739 725 722 7 848 0.73 830 0.80 807 0.87 785 0.92 8 576 619 662 690 9 740 719 716 712 10 594 0.80 623 0.86 678 0.97 684 0.98 11 752 713 710 710 12 728 731 690 685 ¯ψ 0.75 0.82 0.90 0.94 表 6 12M72地螺内力对比分析(S3 = 200.0 mm)
Table 6. Comparative analysis of internal force of 12M72 ground screw (S3 = 200.0 mm)
地螺
编号t = 45 mm t = 55 mm t = 65 mm t = 75 mm N/kN ψo N/kN ψo N/kN ψo N/kN ψo 1 903 1.15 877 1.17 873 1.17 875 1.15 2 1024 1010 1009 1002 3 877 857 854 860 4 960 1.05 975 1.05 952 1.06 948 1.06 5 947 959 960 957 6 849 847 858 864 7 923 1.01 907 1.07 912 1.07 915 1.08 8 937 953 965 970 9 876 874 890 878 10 964 1.07 972 1.10 979 1.12 989 1.13 11 855 882 877 863 12 903 889 874 881 ¯ψ 1.09 1.10 1.10 1.11 表 7 12M72地螺内力对比分析(S3 = 225.0 mm)
Table 7. Comparative analysis of internal force of 12M72 ground screw (S3 = 225.0 mm)
地螺编号 t = 65 mm t = 75 mm t = 65 mm t = 75 mm N/kN ψo N/kN ψo N/kN ψo N/kN ψo 1 950 0.88 924 0.99 903 1.01 910 1.04 2 836 900 905 932 3 943 900 892 888 4 1004 0.86 975 0.95 956 0.99 959 1.02 5 837 892 906 934 6 954 904 882 881 7 981 0.87 966 0.97 949 0.96 956 0.98 8 834 905 890 908 9 935 905 910 900 10 846 0.90 911 1.00 990 1.09 946 1.06 11 925 903 912 887 12 951 914 904 899 ¯ψ 0.88 0.98 1.01 1.02 表 8 12M72地螺内力对比分析(S3 = 250.0 mm)
Table 8. Comparative analysis of internal force of 12M72 ground screw (S3 = 250.0 mm)
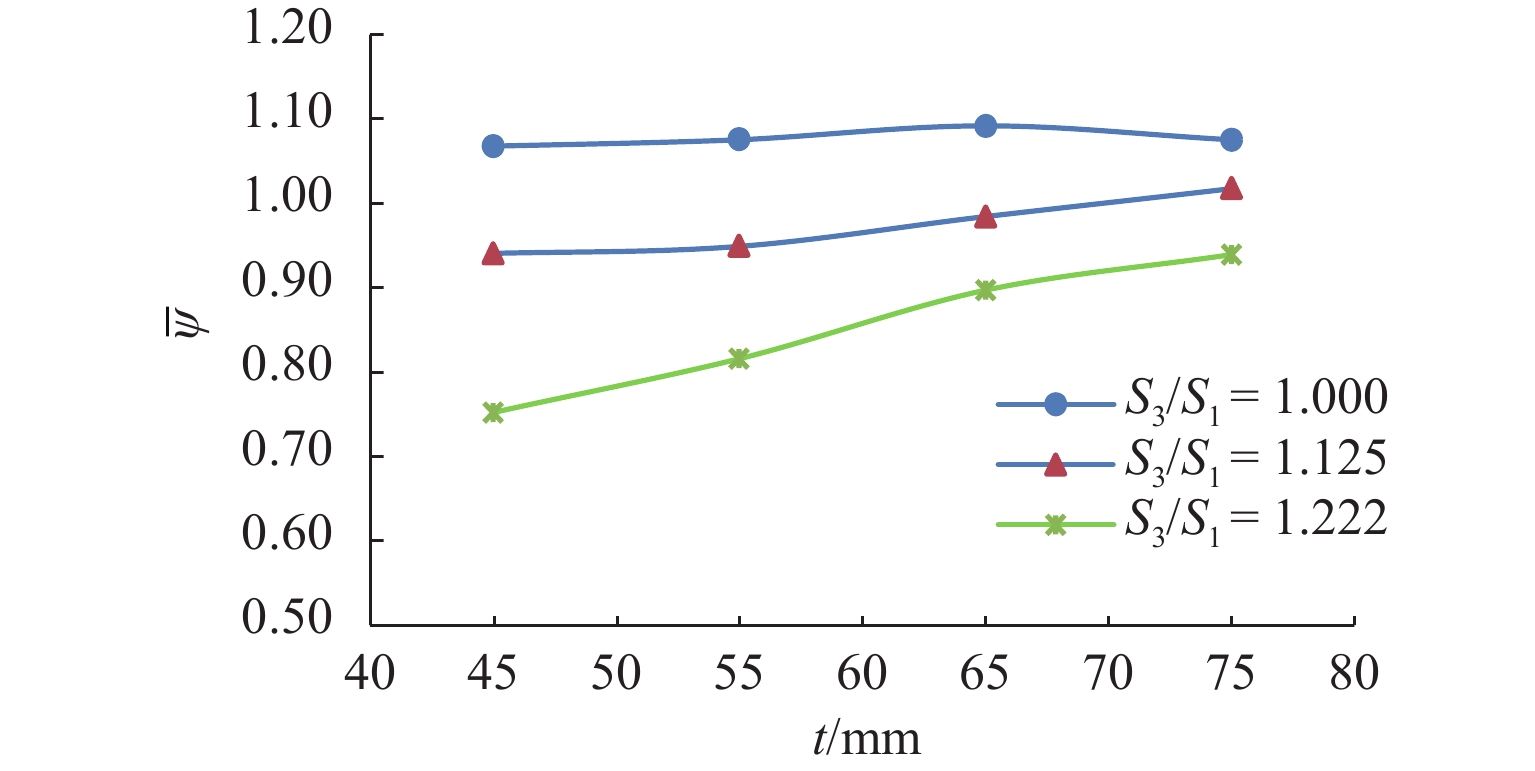
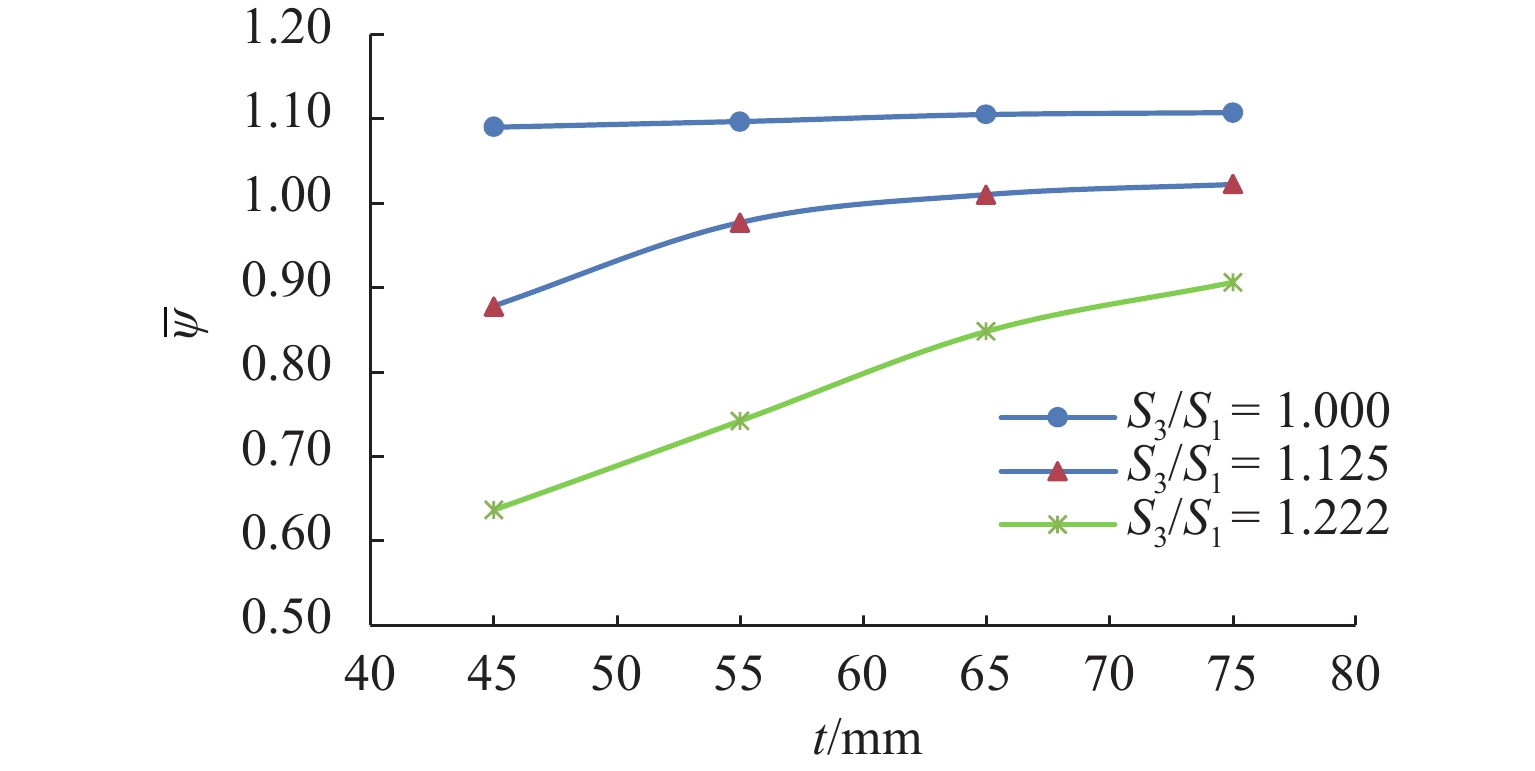
地螺编号 t = 45 mm t = 55 mm t = 65 mm t = 75 mm N/kN ψo N/kN ψo N/kN ψo N/kN ψo 1 1026 0.68 996 0.77 950 0.87 943 0.92 2 692 755 823 855 3 1014 979 942 918 4 1105 0.60 1054 0.70 1038 0.82 1012 0.87 5 637 721 806 844 6 1034 996 926 919 7 1093 0.62 1057 0.72 999 0.85 985 0.91 8 669 726 828 868 9 1048 972 952 924 10 656 0.65 772 0.78 819 0.85 861 0.92 11 1010 984 970 937 12 1023 990 954 937 ¯ψ 0.64 0.74 0.85 0.91 表 9 不均匀分布系数均值¯ψ
Table 9. Mean value of uneven distribution coefficient ¯ψ
地螺模型 t/mm S3/S1 1.000 1.125 1.222 12M64 45 1.07 0.94 0.75 55 1.08 0.95 0.82 65 1.09 0.98 0.90 75 1.08 1.02 0.94 12M72 45 1.09 0.88 0.64 55 1.10 0.98 0.74 65 1.10 1.01 0.85 75 1.11 1.02 0.91 表 10 数值试验参数
Table 10. Numerical test parameters
地螺模型 S1/mm S2/mm S3/mm L/mm t/mm 模型组数/组 12M64 180.0 195.0≥3.0d 180.0,202.5,220.0 150 45,55,65,75 12 225.0≥3.5d 260.0≥4.0d 12M72 200.0 195.0≥3.0d 200.0,225.0,250.0 170 45,55,65,75 12 225.0≥3.5d 260.0≥4.0d 表 11 上拔力计算结果对比
Table 11. Comparison of calculation results of uplift force
地螺型式 ψ 最不利地螺内力/T 内嵌式十二地螺 1.0~1.1 0.083~0.089 八地螺 1.0 0.125 -
[1] 国家能源局. 架空输电线路杆塔结构设计技术规程:DL/T 5486—2020[S]. 北京: 中国电力出版社,2020. [2] 国家能源局. 输电线路杆塔制图和构造规定:DL/T 5442—2020[S]. 北京: 中国电力出版社,2020. [3] 王学明,薛晓敏,文凡,等. 八地脚塔脚板受拉承载力及其设计参数影响分析[J]. 西安交通大学学报,2020,54(3): 169-178.WANG Xueming, XUE Xiaomin, WEN Fan, et al. Research on tensile capacity of eight-bolted baseplate of electricity pylon and the influence parameters[J]. Journal of Xi’an Jiaotong University, 2020, 54(3): 169-178. [4] 杨攀,张雷,孟宪乔,等. 单角钢八地脚螺栓的受力分析及其塔脚板厚度设计[J]. 南昌大学学报(工科版),2016,38(3): 240-244. doi: 10.3969/j.issn.1006-0456.2016.03.007YANG Pan, ZHANG Lei, MENG Xianqiao, et al. Stress analysis of single angle eight foundation bolts and its design of bottom plate thickness[J]. Journal of Nanchang University (Engineering & Technology), 2016, 38(3): 240-244. doi: 10.3969/j.issn.1006-0456.2016.03.007 [5] 袁红丽. 特高压输电塔塔座板承载力研究及地震响应分析[D]. 西安: 西安建筑科技大学,2019. [6] 何松洋,韩大刚,蒋锐,等. 输电塔塔脚板式靴板连接计算方法[J]. 土木工程与管理学报,2021,38(4): 127-132,146. doi: 10.3969/j.issn.2095-0985.2021.04.019HE Songyang, HAN Dagang, JIANG Rui, et al. Calculation method on shoe plate connection of transmission tower[J]. Journal of Civil Engineering and Management, 2021, 38(4): 127-132,146. doi: 10.3969/j.issn.2095-0985.2021.04.019 [7] 付庭聪,肖奉英,孟凯,等. 塔脚板劲板尺寸对底板传递力分配比的影响[J]. 南昌大学学报(工科版),2022,44(3): 246-253. doi: 10.3969/j.issn.1006-0456.2022.03.007FU Tingcong, XIAO Fengying, MENG Kai, et al. Distribution ratio of tower foot stiffener plate size to bottom plate transfer force[J]. Journal of Nanchang University (Engineering & Technology), 2022, 44(3): 246-253. doi: 10.3969/j.issn.1006-0456.2022.03.007 [8] 蒋童,徐海潮,张树林,等. 塔脚板结构强度与底板接触应力分析[J]. 南昌大学学报(工科版),2021,43(4): 347-353. doi: 10.3969/j.issn.1006-0456.2021.04.007JIANG Tong, XU Haichao, ZHANG Shulin, et al. Analysis of tower foot structure strength and bottom plate contact stress[J]. Journal of Nanchang University (Engineering & Technology), 2021, 43(4): 347-353. doi: 10.3969/j.issn.1006-0456.2021.04.007 [9] 周文杰. 输电线路塔脚板的受力分析及其结构优化研究[D]. 南昌: 南昌大学,2019. [10] 俞登科,任吉华,段松涛,等. 基于区格法的输电塔有加劲塔脚板强度分析[J]. 电力勘测设计,2017(2): 42-45.YU Dengke, REN Jihua, DUAN Songtao, et al. Strength analysis of tower footing plate with stiffening in transmission tower based on lattice method[J]. Electric Power Survey & Design, 2017(2): 42-45. [11] 蒋童. 输电铁塔塔脚板结构强度与底板接触应力分布的研究[D]. 南昌: 南昌大学,2022. [12] 马瑞君,吴森坤,吕健,等. 输电铁塔十二颗地脚螺栓塔脚节点设计方法研究[J]. 工业建筑,2019,49(4): 44-51.MA Ruijun, WU Senkun, LYU Jian, et al. Research on design method of twelve-bolted leg joint of transmission tower[J]. Industrial Construction, 2019, 49(4): 44-51. [13] 向越,马海云,刘洪昌,等. 一种组合角钢塔圆形塔脚板布置结构:CN215717694U[P]. 2022-02-01. [14] KENNEDY G GOODCHILD C. Practical yield line design[M]. London: British Cement Association, 2003. [15] 沈聚敏,王传志,江见鲸. 钢筋混凝土有限元与板壳极限分析[M]. 北京: 清华大学出版社,1993. [16] 王稳,郝鑫,曹岳,等. 基于屈服线理论的塔脚板上拔承载力计算方法[C]//2021年工业建筑学术交流会论文集(上册). 北京: 国核电力规划设计研究院有限公司,2021:254-258,367. [17] 中华人民共和国住房和城乡建设部. 钢结构设计标准:GB 50017—2017[S]. 北京: 中国建筑工业出版社,2017. [18] 何松洋,蒋锐,辜良雨,等. 输电塔挂线角钢节点理论计算方法研究[J]. 建筑钢结构进展,2022,24(4): 82-91.HE Songyang, JIANG Rui, GU Liangyu, et al. Theoretical calculation method of angle steel joint of transmission tower[J]. Progress in Steel Building Structures, 2022, 24(4): 82-91. [19] 杨昌. 输电铁塔连接节点螺栓群力分配与螺栓布置形式和预拉力的关系研究[D]. 南昌: 南昌大学,2017. [20] 国家能源局. 输电线路杆塔及电力金具用热浸镀锌螺栓与螺母:DL/T 284—2021[S]. 北京: 中国电力出版社,2021. -





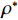
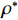

 下载:
下载:
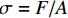
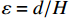




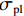
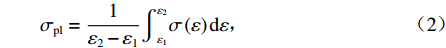
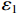
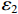


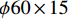
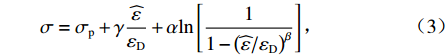
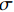
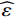
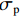
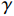
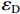
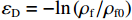
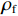

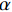

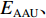










 下载:
下载: