Axial Compression Test and Bearing Capacity Design Method of Cold-Formed Steel with Unequal-Leg Lipped Angles
-
摘要:
为研究轴心受压冷弯薄壁不等肢卷边角钢屈曲性能和承载力设计方法,采用试验和有限元程序分析其屈曲性能和极限承载力,并基于直接强度法提出承载力设计建议方法. 首先,开展32根不同截面、长细比和宽厚比的冷弯薄壁不等肢卷边角钢轴压试验;随后,采用ABAQUS有限元软件对冷弯薄壁不等肢卷边角钢在不同宽厚比、肢宽比、长细比等条件下的屈曲性能和承载力进行参数化分析;最后,基于试验和有限元分析结果提出轴心受压冷弯薄壁不等肢卷边角钢构件承载力计算的修正直接强度法公式. 结果表明:宽厚比较小,试件易发生弯扭屈曲,宽厚比较大,试件易发生局部屈曲(或弯扭)和局部相关屈曲;构件极限承载力随长细比增大而降低,随着宽厚比的增大,承载力增长趋势逐渐减缓;提出的修正直接强度法计算轴心受压冷弯薄壁不等肢卷边角钢构件承载力的可靠度指标均值均大于3.2,建议方法准确且安全可靠.
Abstract:To study the buckling performance and bearing capacity design method of the cold-formed thin-walled steel (CFTWS) with unequal-leg lipped angles under axial compression, the tests were conducted, and a finite element program was used to analyze the buckling performance and ultimate bearing capacity. Based on the direct strength method, the bearing capacity design method was presented. Firstly, the 32 CFTWS with unequal-leg lipped angles with different sections, slenderness ratios, and width-to-thickness ratios were tested under axial compression. Then, ABAQUS finite element software was used to perform parametric analysis on the buckling performance and bearing capacity of CFTWS with unequal-leg lipped angles with different width-to-thickness ratios, width ratios of legs, and slenderness ratios. Finally, the modified direct strength method for calculating the bearing capacity of CFTWS with unequal-leg lipped angles under axial compression was proposed based on the results of the test and finite element analysis. The results show that the specimens with a small width-to-thickness ratio are prone to flexural-torsional buckling, while the specimens with a large width-to-thickness ratio are prone to the local buckling or buckling related to flexural-torsional buckling and local buckling. The ultimate bearing capacity of the specimens decreases with the increase in the slenderness ratio, and the increase amplitude of the bearing capacity slows down with the increase in the width-to-thickness ratio. The reliability analysis shows that the reliability indexes of the bearing capacity of the CFTWS with unequal-leg lipped angles under axial compression calculated using the modified direct strength method are greater than 3.2, indicating that the proposed method is accurate and reliable.
-
随着我国冷弯技术的快速发展,冷弯薄壁型钢凭借其轻质高强、利用率高的优点,在土木工程中的应用日益广泛[1]. 而冷弯薄壁不等肢卷边角钢由于截面简单、工业化程度高、安装简易,常被应用于可拆式箱式房屋以及冷弯型钢结构房屋中.
Adluri等[2]对铰接细长轴压角钢柱弯曲屈曲进行试验研究,表明由于局部屈曲引起有效截面形心的偏移,导致荷载偏心,引起附加弯矩,承载力计算时需考虑其影响;Dinis等[3]也指出,采用北美规范[4]计算角钢柱承载力,仅需考虑L/
1000 的偏心,其中,L为试件几何长度;Young[5]对固接冷弯平板角钢柱进行轴压试验,结果表明,承载力设计应只考虑弱轴的弯曲屈曲,而忽略弹性扭转屈曲和弯扭屈曲以及偏心引起的附加弯矩;De Barros Chodraui等[6]通过有限条法对轴心受压冷弯角钢柱进行分析,表明板件局部屈曲和整体扭转屈曲是一致的;Silvestre等[7]通过理论和有限元分析表明,固接和销轴连接的短、中长等肢角钢柱的屈曲和后屈曲有一定的不同;Ellobody等[8]以及Young等[9]分别对轴心受压平板角钢和卷边角钢屈曲性能进行计算分析,表明北美规范[4]对宽厚比较大构件承载力计算较为保守,而对宽厚比较小构件计算承载力偏于不安全;在此基础上,Young等[10]进行了屈曲强度450 MPa和550 MPa不等肢卷边角钢轴压试验,试件发生了弯扭屈曲以及局部和弯扭屈曲的相关屈曲,结果表明,北美规范[4]偏于保守;Shifferaw等[11]对固接冷弯薄壁等肢角钢柱整体屈曲性能进行理论和有限元分析,表明构件发生整体弯扭屈曲时表现出较为显著的屈曲后强度;Zhang等[12]采用试验和数值模拟研究S690高强角钢柱的弯扭屈曲性能和承载力,表明设计规范均给出了过于保守和分散的弯扭屈曲承载力;姚行友等[13]等对冷弯不等肢复杂卷边角钢进行轴压试验和有限元参数分析,提出考虑整体屈曲后强度的直接强度法修正公式;周宜松[14]对复杂卷边角钢柱进行轴压试验,发现试件越长越容易发生整体失稳,并提出适用复杂卷边不等肢角钢的直接强度法建议公式;徐步洲[15]通过对不等肢卷边角钢进行试验和有限元分析表明,中等长度柱易发生畸变和局部的相关屈曲,分肢宽厚比较大的卷边发生明显的畸变屈曲现象.尽管国内外学者对轴心受压冷弯薄壁型角钢进行了大量研究,但针对不等肢卷边角钢受力性能及设计方法的研究仍相对较少,我国现行国家规范《冷弯薄壁型钢结构技术规范》[16]也缺乏对双轴不对称冷弯薄壁截面构件承载力的设计方法. 鉴于此,本文对32根冷弯薄壁不等肢卷边角钢轴压试件的屈曲性能和极限承载力进行试验研究. 基于验证的有限元模型,分析长细比、宽厚比、肢宽比等参数对其屈曲性能和极限承载力的影响. 基于试验和有限元分析结果提出轴心受压冷弯薄壁不等肢卷边角钢构件承载力计算直接强度法的修正公式.
1. 试验概况
1.1 试件设计
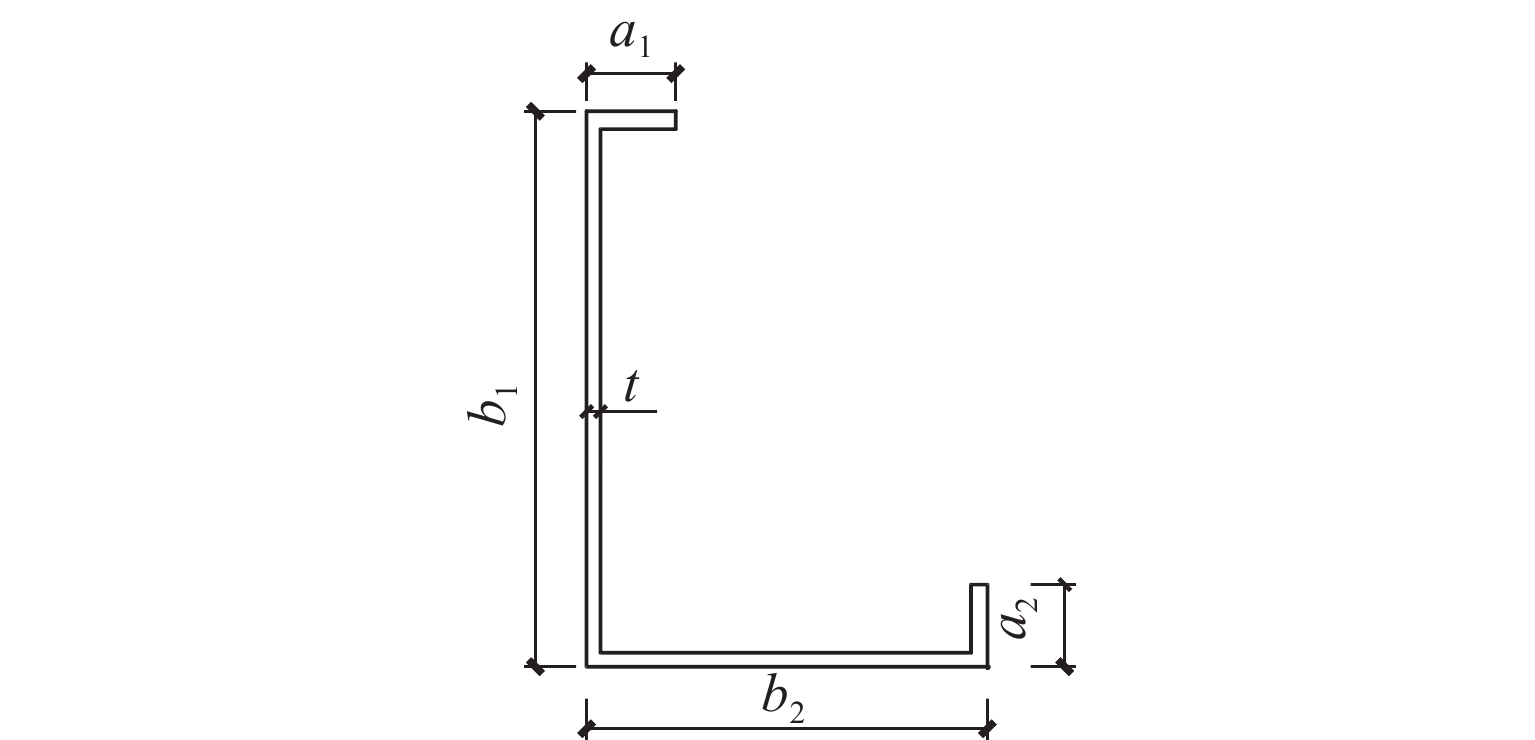
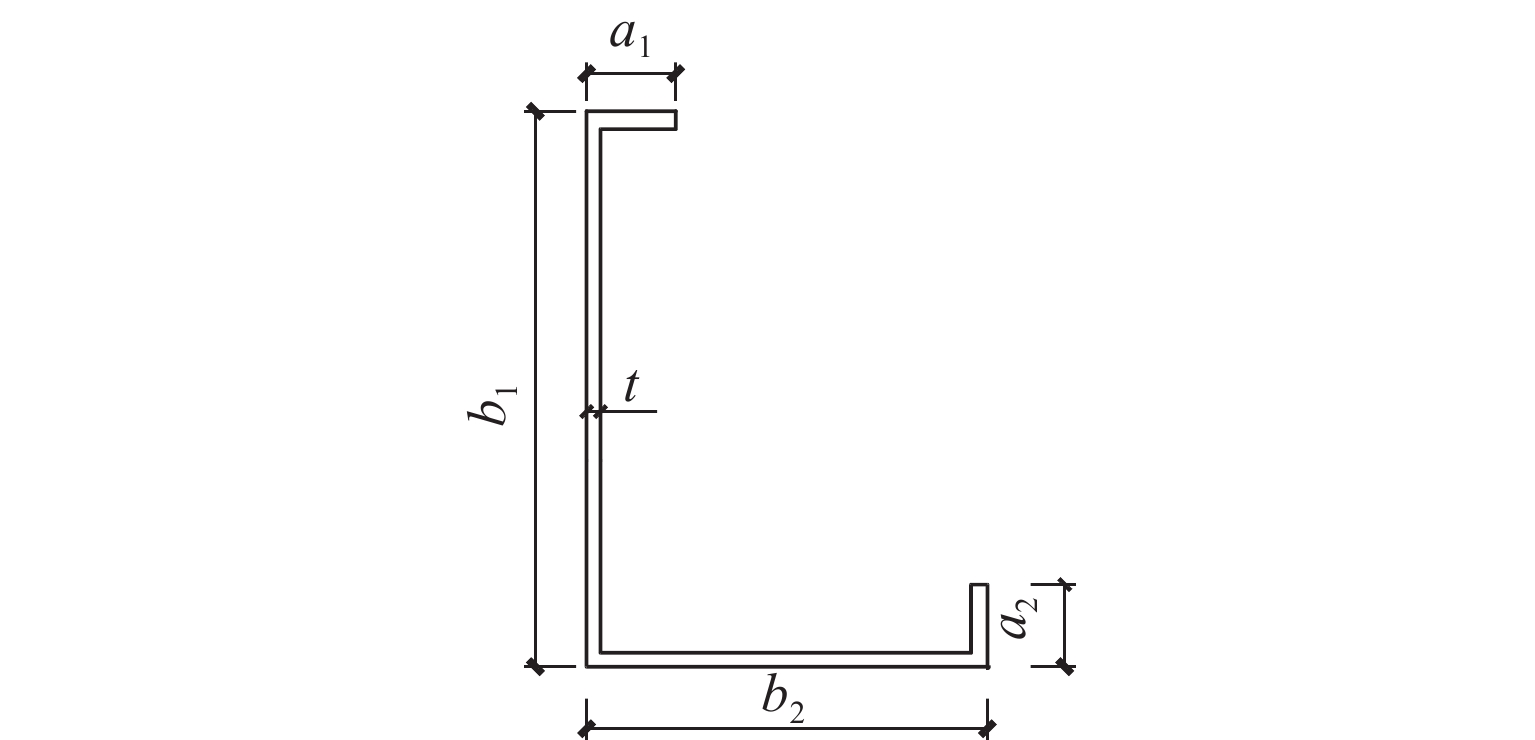
本试验共设计32根冷弯薄壁不等肢卷边角钢试件,试件板材为LQ550镀锌钢板,名义厚度为2 mm,截面形式如图1所示. 图中:b1、b2分别为构件长肢和短肢的宽度,a1、a2为对应卷边的宽度,t为板厚,试件编号形式为LL4030-400-1,其中:LL表示不等肢卷边角钢,40、30分别表示长肢和短肢宽度,400表示角钢长度,1表示相同试件序号. 试件名义几何尺寸和实测尺寸如表1和表2所示.
表 1 试件名义几何尺寸Table 1. Nominal geometric dimension of specimens试件编号 bl/mm b2/mm al/mm a2/mm t/mm b1/t b2/t LL4030 40 30 15 15 2 20 15 LL6040 60 40 20 20 2 30 20 LL9060 90 60 20 20 2 45 30 LL12080 120 80 24 24 2 60 40 表 2 试件实测几何尺寸Table 2. Measured geometric dimensions of specimens试件编号 bl/mm b2/mm al/mm a2/mm t/mm 长度 L/mm 长细比 λ LL4030-400-1 42.13 31.43 15.97 15.54 1.98 400.00 39.19 LL4030-400-2 41.51 31.45 15.56 16.34 1.98 399.27 39.88 LL4030-900-1 42.00 31.61 15.96 14.84 1.99 900.00 87.28 LL4030-900-2 42.25 31.05 15.14 15.79 2.00 900.00 89.45 LL4030-1500-1 41.98 31.48 15.99 15.28 1.97 1498.37 147.14 LL4030-1500-2 41.93 31.42 15.93 15.95 2.00 1499.33 146.65 LL4030-2100-1 42.07 31.32 15.60 15.96 1.98 2101.17 206.51 LL4030-2100-2 42.20 31.59 15.52 15.66 1.98 2101.27 206.93 LL6040-400-1 61.68 41.48 21.63 19.48 1.98 400.00 29.54 LL6040-400-2 61.35 41.52 21.17 21.00 1.97 399.00 29.40 LL6040-900-1 62.05 41.60 21.45 19.37 1.98 900.00 66.46 LL6040-900-2 62.16 41.63 21.54 19.61 2.09 900.00 68.25 LL6040-1500-1 62.10 41.64 21.06 20.48 1.99 1499.43 110.40 LL6040-1500-2 61.80 41.76 21.35 20.98 2.07 1499.27 111.54 LL6040-2100-1 62.24 41.37 21.32 19.86 1.91 2101.10 152.65 LL6040-2100-2 61.44 41.91 20.92 20.47 1.98 2100.00 154.12 LL9060-400-1 91.80 61.41 20.25 20.02 1.97 400.00 22.15 LL9060-400-2 91.92 61.31 20.12 20.50 1.97 399.50 22.16 LL9060-900-1 91.51 61.43 19.90 21.05 1.99 900.00 49.79 LL9060-900-2 91.90 61.89 20.67 20.19 2.00 900.00 49.39 LL9060-1500-1 91.50 62.59 20.51 20.88 2.00 1498.90 81.56 LL9060-1500-2 91.43 62.42 21.08 19.88 1.97 1499.10 81.69 LL9060-2100-1 92.07 61.52 20.99 19.69 1.98 2100.00 115.66 LL9060-2100-2 91.60 61.94 21.83 21.01 2.00 2101.03 113.94 LL12080-400-1 121.53 81.77 24.92 23.98 1.98 400.00 16.95 LL12080-400-2 121.30 81.82 24.19 24.22 1.98 399.27 17.00 LL12080-900-1 122.30 81.71 25.11 24.05 1.97 900.00 38.10 LL12080-900-2 121.87 81.95 23.68 24.90 1.97 900.00 38.23 LL12080-1500-1 121.97 82.20 24.71 24.17 2.00 1498.22 63.34 LL12080-1500-2 122.48 81.87 24.95 24.70 1.97 1499.13 63.33 LL12080-2100-1 122.79 81.89 23.76 24.03 1.98 2101.17 89.38 LL12080-2100-2 121.04 82.24 24.71 24.49 1.97 2100.14 88.61 1.2 材性试验
材性试验共设计3个标准试样,所有试样均来自同一母材,根据《金属材料拉伸试验室温试验第1部分:室温试验方法》[17]中的规定方法进行拉伸试验.
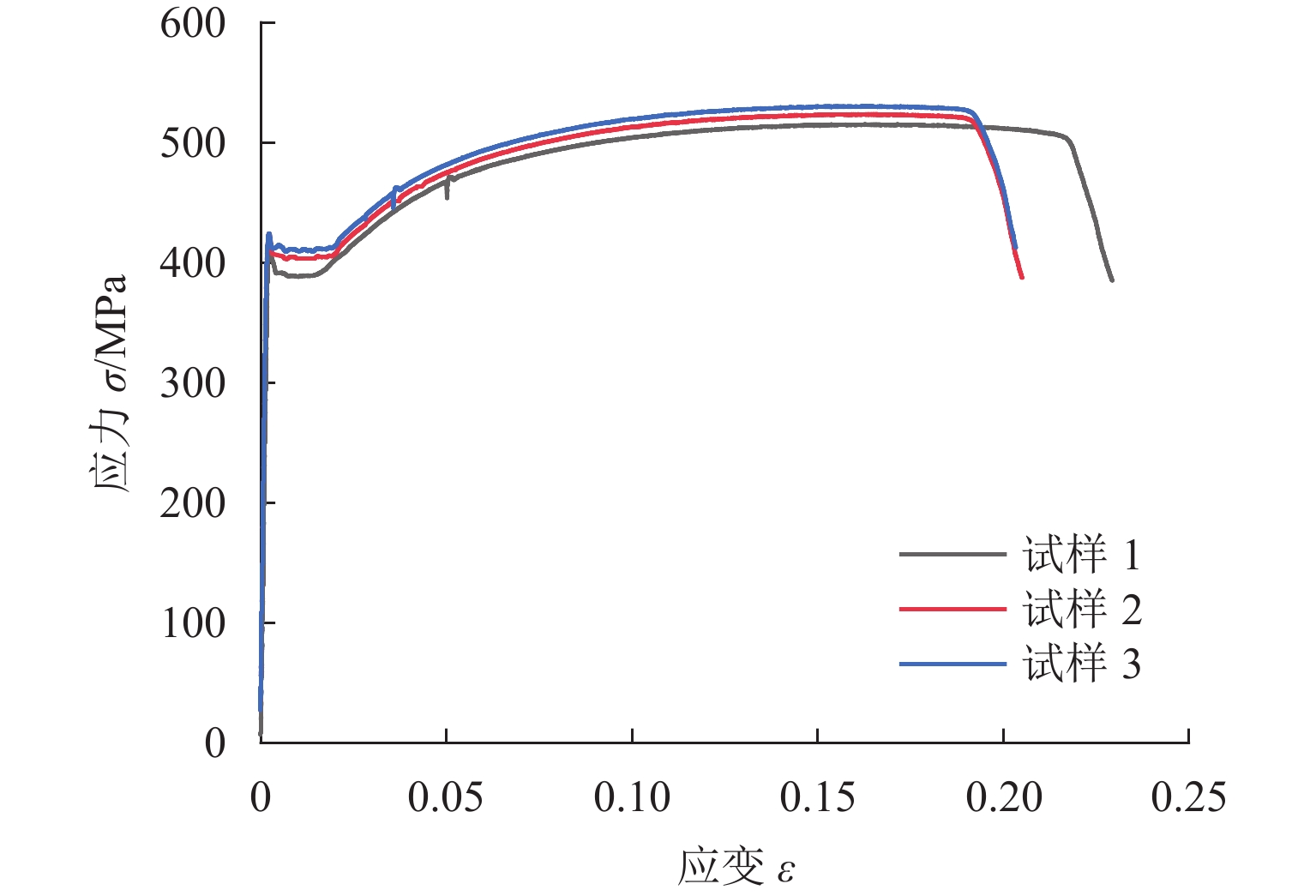
3个标准试样材性试验应力-应变曲线如图2所示. 得到屈服强度fy=403.0 MPa,极限强度fu=522.8 MPa,弹性模量E=2.11 × 1011 Pa,断后伸长率δ=31.3%.
1.3 初始缺陷
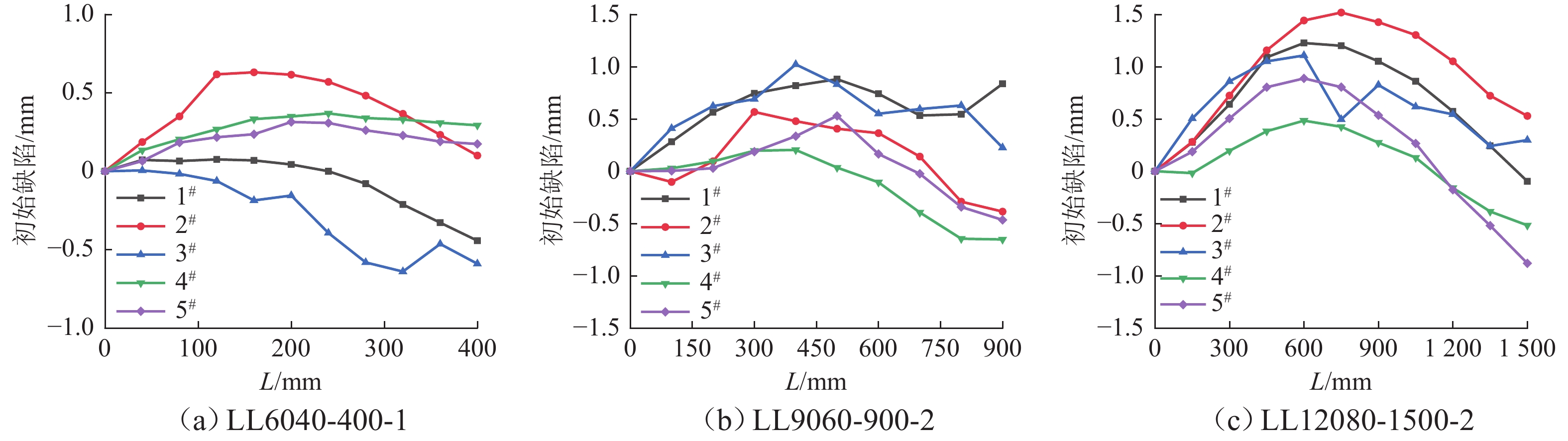
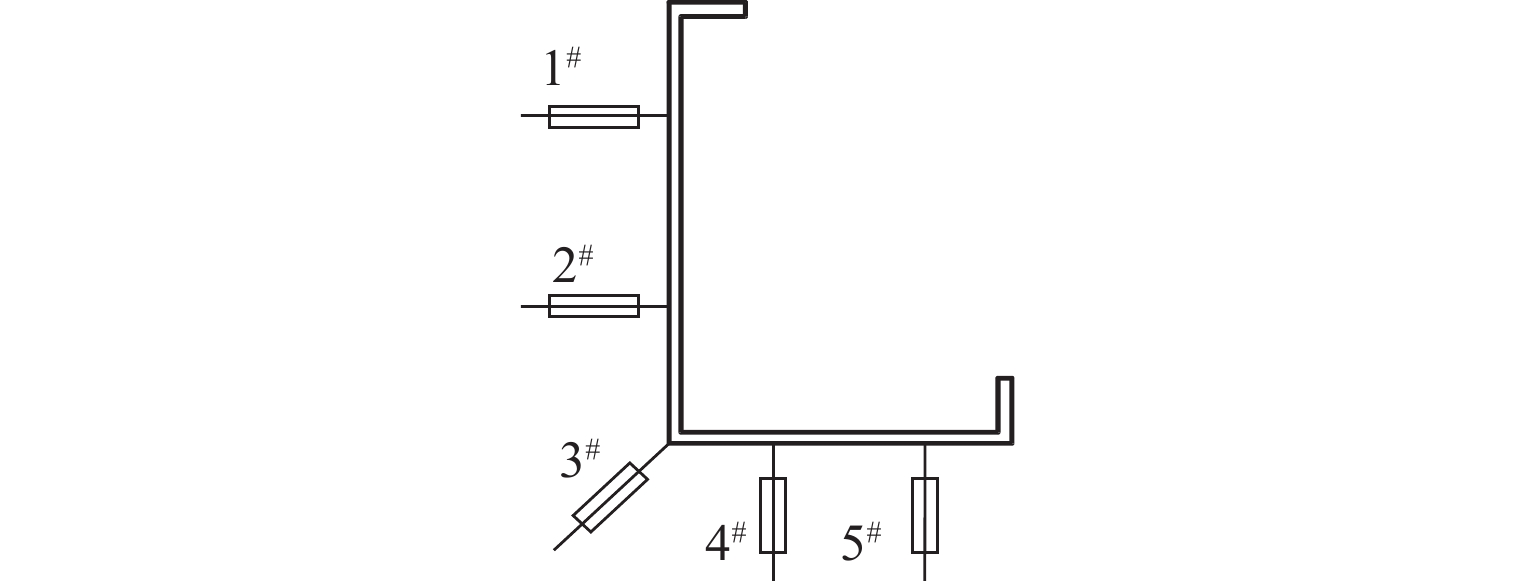
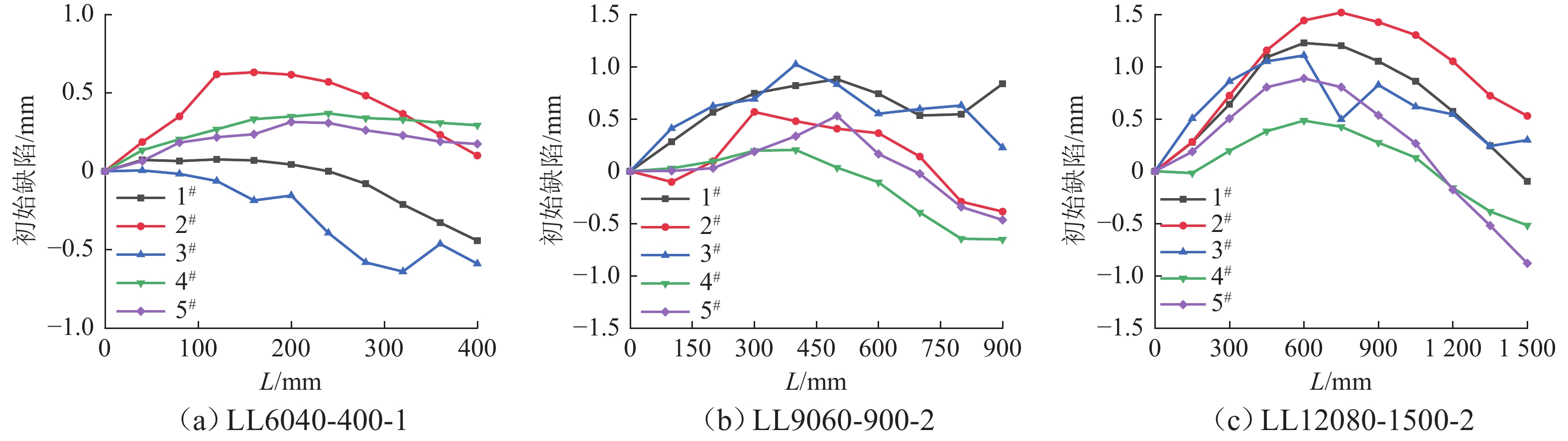
试验前采用千分表对所有试件的初始几何缺陷进行量测. 试件纵向初始缺陷量测位置如图3所示. 外凸变形取值为正,内凹变形取值为负,1#、2#、4#、5# 千分表测量试件肢宽边缘10 mm处沿纵向分肢初始局部屈曲缺陷,3# 千分表量测试件整体弯曲初始缺陷. 部分试件沿纵向初始缺陷如图4所示,其他试件初始几何缺陷与其基本一致,并无明显的分布规律. 表3给出所有试件初始几何缺陷测量结果的最大值. 表中:ΔLmax、ΔGmax分别为局部屈曲缺陷与整体屈曲缺陷;Pt、PFE、PD、PMD、PY分别为试件试验极限承载力、试件有限元分析极限承载力、北美规范直接强度法计算极限承载力、修正直接强度法计算极限承载力、文献[18]建议修正公式计算极限承载力;F、L、D、FT分别表示弯曲屈曲、局部屈曲、畸变屈曲和弯扭屈曲. 由表3可知,试件的局部屈曲缺陷值均大于整体屈曲缺陷值,试件的整体初始缺陷均小于L/
1000 .表 3 试验和有限元分析角钢屈曲模式和承载力对比Table 3. Comparisons of buckling modes and bearing capacities of steel angles between test and finite element analysis试件编号 屈曲模式 ΔLmax/
mmΔGmax/
mmPt/kN PFE/kN PD/kN PMD/kN PY/kN Pt/PY Pt/ PFE Pt/ PD Pt/PMD 试验 有限元 一阶模态 LL4030-400-1 FT FT F 0.49 0.28 79.66 83.17 83.77 74.38 72.51 1.0986 0.9578 0.9509 1.0710 LL4030-400-2 FT FT F 0.41 0.34 79.38 82.66 83.67 73.51 73.41 1.0813 0.9603 0.9488 1.0799 LL4030-900-1 FT FT F 1.30 0.83 50.68 53.93 56.47 50.71 27.68 1.8312 0.9397 0.8974 0.9995 LL4030-900-2 FT FT F 1.54 0.77 52.72 54.70 56.90 50.97 27.97 1.8852 0.9638 0.9265 1.0342 LL4030-1500-1 FT FT F 1.30 0.38 40.70 40.93 40.36 42.15 23.01 1.7689 0.9944 1.0085 0.9655 LL4030-1500-2 FT FT F 1.14 0.17 40.98 41.52 41.89 43.75 23.88 1.7159 0.9869 0.9783 0.9366 LL4030-2100-1 FT FT F 1.84 0.08 30.52 31.19 24.48 25.57 13.96 2.1867 0.9786 1.2467 1.1936 LL4030-2100-2 FT FT F 1.10 0.09 29.26 31.35 24.50 25.59 13.97 2.0948 0.9335 1.2343 1.1434 LL6040-400-1 L L L 0.67 0.46 103.70 105.82 102.94 95.81 119.12 0.8706 0.9800 1.0074 1.0824 LL6040-400-2 L L L 0.75 0.31 102.46 105.26 105.33 96.41 121.73 0.8417 0.9734 0.9728 1.0628 LL6040-900-1 FT FT F 1.08 0.63 83.69 85.39 94.17 76.27 52.34 1.5989 0.9800 0.9886 1.0973 LL6040-900-2 FT FT F 1.17 0.74 83.62 84.70 98.45 80.28 54.25 1.5414 0.9872 0.9494 1.0416 LL6040-1500-1 FT FT F 1.15 0.60 59.96 61.98 74.21 61.37 42.31 1.4172 0.9673 0.9480 0.9769 LL6040-1500-2 FT FT F 1.42 0.43 60.82 63.31 78.23 64.30 44.60 1.3638 0.9607 0.9775 0.9459 LL6040-2100-1 FT FT F 1.17 0.24 48.66 50.69 41.59 43.44 23.71 2.0519 0.9600 1.1699 1.1201 LL6040-2100-2 FT FT F 1.42 1.08 49.94 50.96 42.99 44.90 24.51 2.0376 0.9800 1.1617 1.1122 LL9060-400-1 L L L 0.99 0.35 126.76 129.35 104.56 124.03 153.51 0.8257 0.9800 1.2123 1.0220 LL9060-400-2 L L L 0.57 0.57 125.71 129.60 106.03 124.39 155.32 0.8094 0.9700 1.1855 1.0106 LL9060-900-1 FT + L FT + L L 1.12 0.71 94.42 96.00 108.68 101.84 68.10 1.3865 0.9835 0.9688 0.9271 LL9060-900-2 FT + L FT + L L 1.02 0.92 95.60 97.20 107.99 102.07 67.07 1.4253 0.9835 0.9853 0.9366 LL9060-1500-1 FT + L FT + L L 0.79 0.71 77.34 80.36 90.86 94.90 51.80 1.4929 0.9625 0.9512 0.8149 LL9060-1500-2 FT + L FT + L L 0.88 0.83 77.04 78.87 85.54 89.34 48.77 1.5797 0.9768 0.9006 0.8623 LL9060-2100-1 D D F 1.22 0.90 59.95 61.37 48.74 50.90 27.79 2.1574 0.9768 1.2300 1.1776 LL9060-2100-2 D D F 1.08 0.77 61.51 63.42 54.77 57.20 31.22 1.9701 0.9700 1.2232 1.0754 LL12080-400-1 L L L 0.59 0.22 147.54 150.67 117.71 146.76 224.28 0.6578 0.9792 1.2535 1.0053 LL12080-400-2 L L L 0.58 0.14 146.78 150.41 117.29 146.49 223.06 0.6580 0.9759 1.2515 1.0020 LL12080-900-1 FT + L FT + L L 1.20 0.56 136.59 138.37 117.55 118.57 105.70 1.2923 0.9872 1.1620 1.1520 LL12080-900-2 FT + L FT + L L 1.52 1.11 131.36 138.86 118.35 118.60 106.10 1.2380 0.9460 1.1099 1.1075 LL12080-1500-1 FT + L FT + L L 1.20 1.56 97.14 98.99 116.57 104.35 81.31 1.1948 0.9813 0.8334 0.9309 LL12080-1500-2 FT + L FT + L L 1.52 1.11 98.56 98.19 116.67 102.58 83.41 1.1817 1.0038 0.8447 0.9608 LL12080-2100-1 FT + L FT + L L 1.44 1.42 85.43 85.91 73.43 76.89 41.97 2.0354 0.9948 1.2634 1.1110 LL12080-2100-2 FT + L FT + L L 1.45 1.54 85.82 86.48 76.31 80.96 44.19 1.9419 0.9926 1.2246 1.0600 均值 1.4760 0.9741 1.1246 1.0318 方差 0.2060 0.0002 0.0227 0.0077 变异系数 0.1395 0.0002 0.0201 0.0074 1.4 加载装置和测点布置
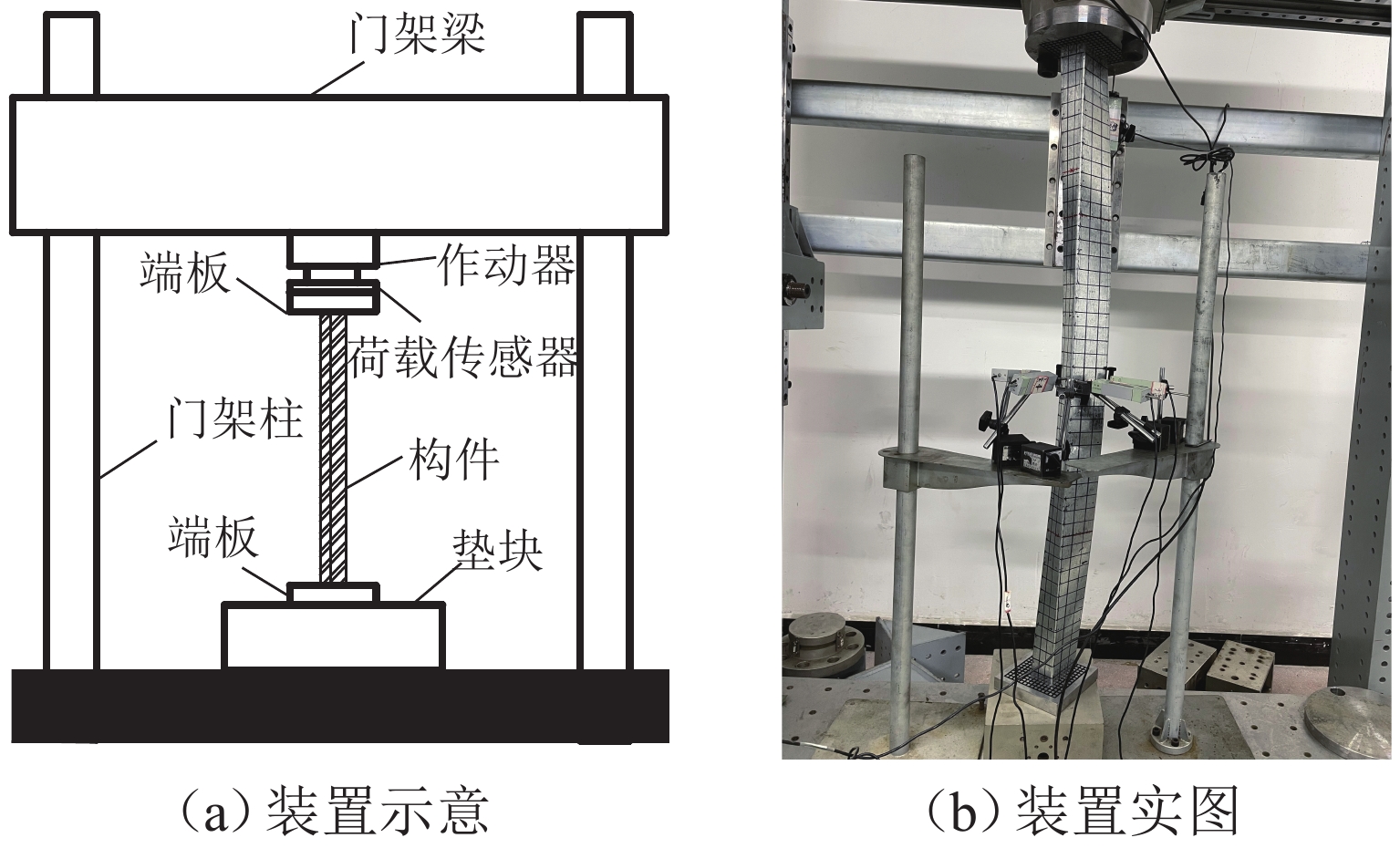
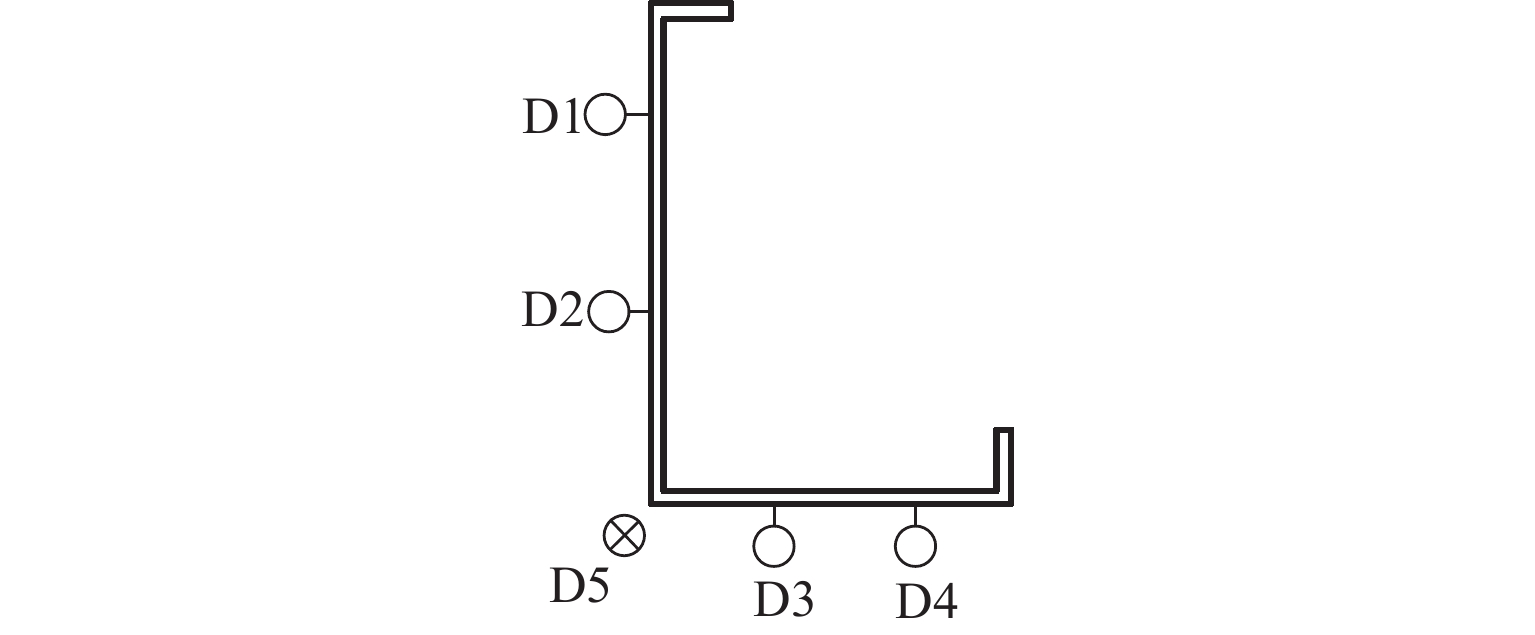
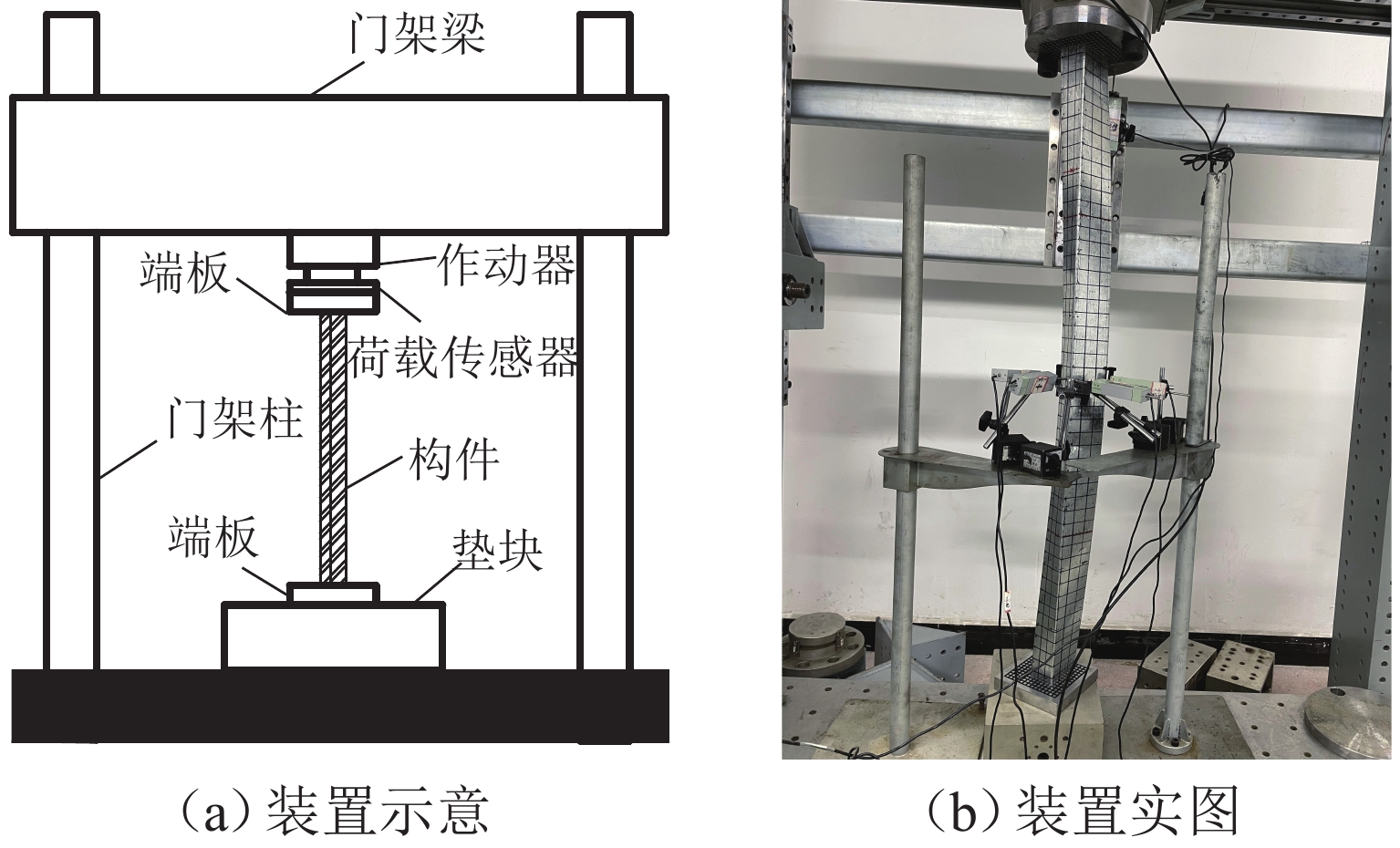
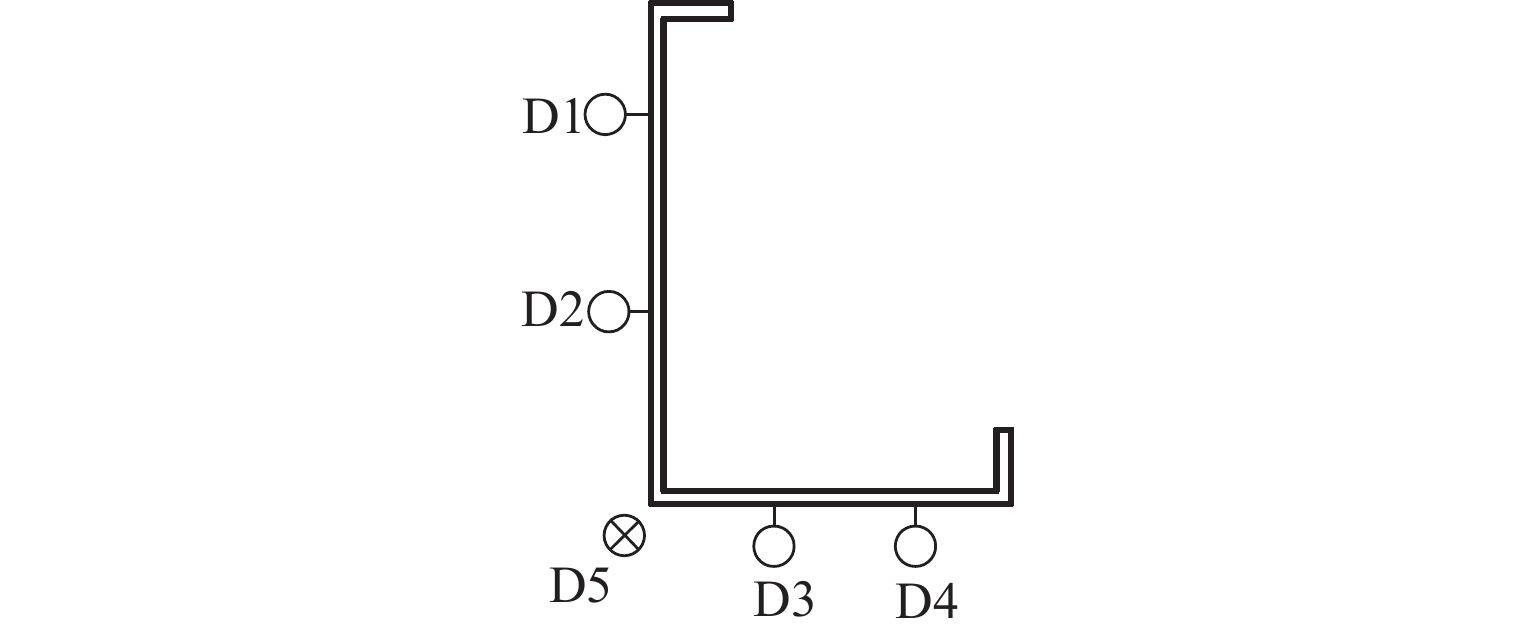
试验采用自平衡反力系统施加竖向荷载,YJ16静态应变位移采集系统采集数据. 加载端板和底板设10 mm深凹槽,试件插入凹槽后采用垫片将凹槽缝隙填满嵌实以保证试件端部固结. 试验加载装置如图5所示. 采用分级加载的加载方式,每级加载后暂停1 min;当试件发生屈曲或承载力开始下降时,加载速率减半;当试件承载力下降至极限承载力的75%或完全破坏,结束加载. 试件位移计布置如图6所示,D1、D2、D3、D4 4个位移计分别布置在试件中截面处,以量测试件跨中的扭转变形,在试件加载端端部布置位移计D5测量竖向位移.
2. 试验结果及分析
2.1 试验过程和试验现象
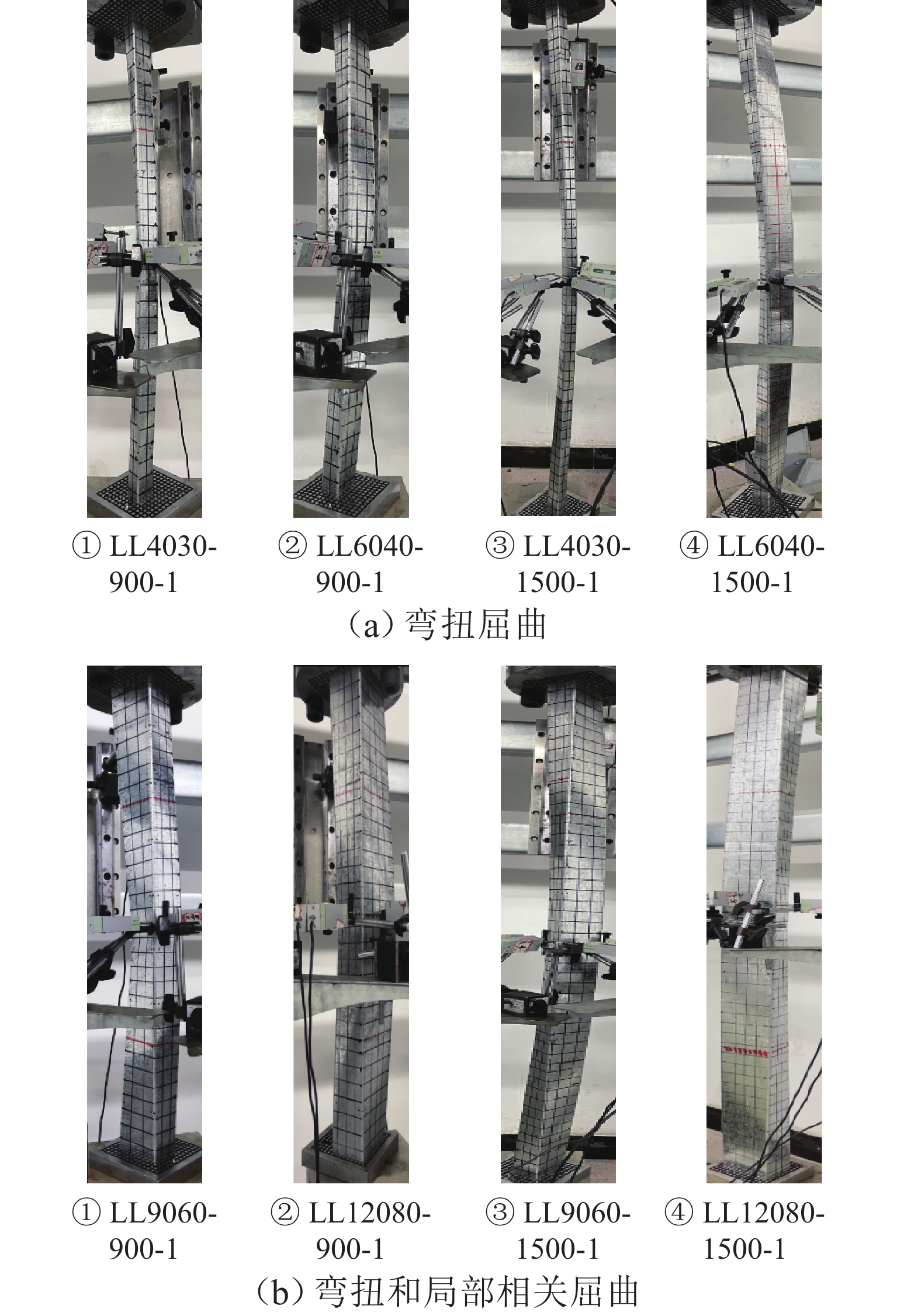
2.1.1 长度为400 mm角钢柱
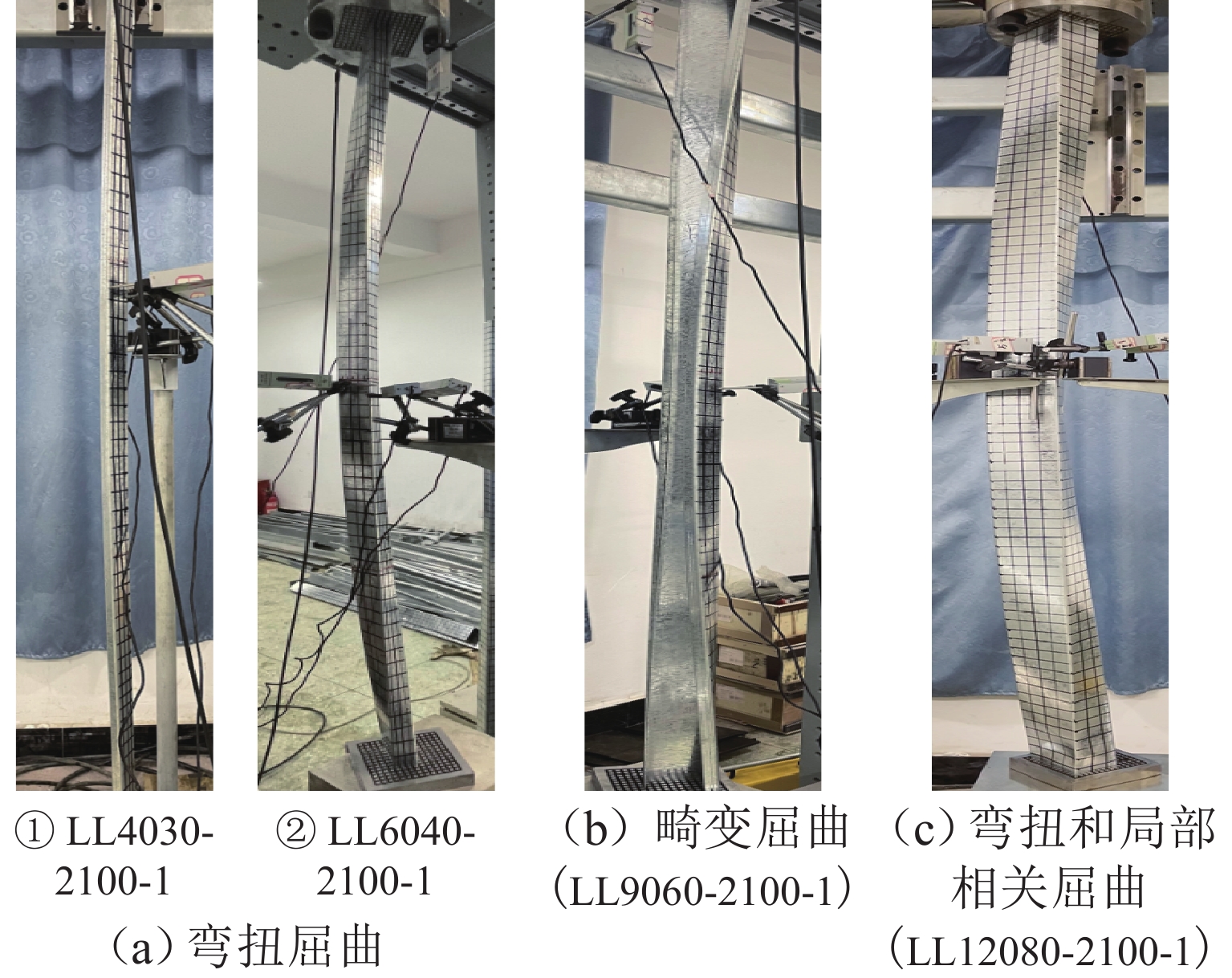
长度为400 mm的不等肢角钢柱屈曲模式如图7所示. 试件在加载初期变形不明显,随着荷载逐渐增大,b2/t=15的试件分肢出现扭转变形且伴有弯曲,达到破坏荷载时长肢扭转变形明显,构件最终表现为弯扭屈曲模式(图7(a)). b2/t=20,30,40的试件出现了明显的局部屈曲,最终表现为塑性折曲破坏(图7(b)).
2.1.2 长度为900、
1500 mm角钢柱长度为900、
1500 mm的试件屈曲模式如图8所示. 在加载初期变形不明显,随着荷载逐渐增大,b2/t=15,20的试件开始整体弯曲,且分肢沿短肢方向出现向内扭转,达到破坏荷载时长短肢出现明显扭转,构件最终表现为弯扭屈曲(图8(a)),b2/t=30,40的试件出现双肢扭转变形且发生整体弯曲,随着长度的增加弯扭现象愈明显,分肢出现局部屈曲,最终表现为整体弯扭和局部相关屈曲(图8(b)).2.1.3 长度为
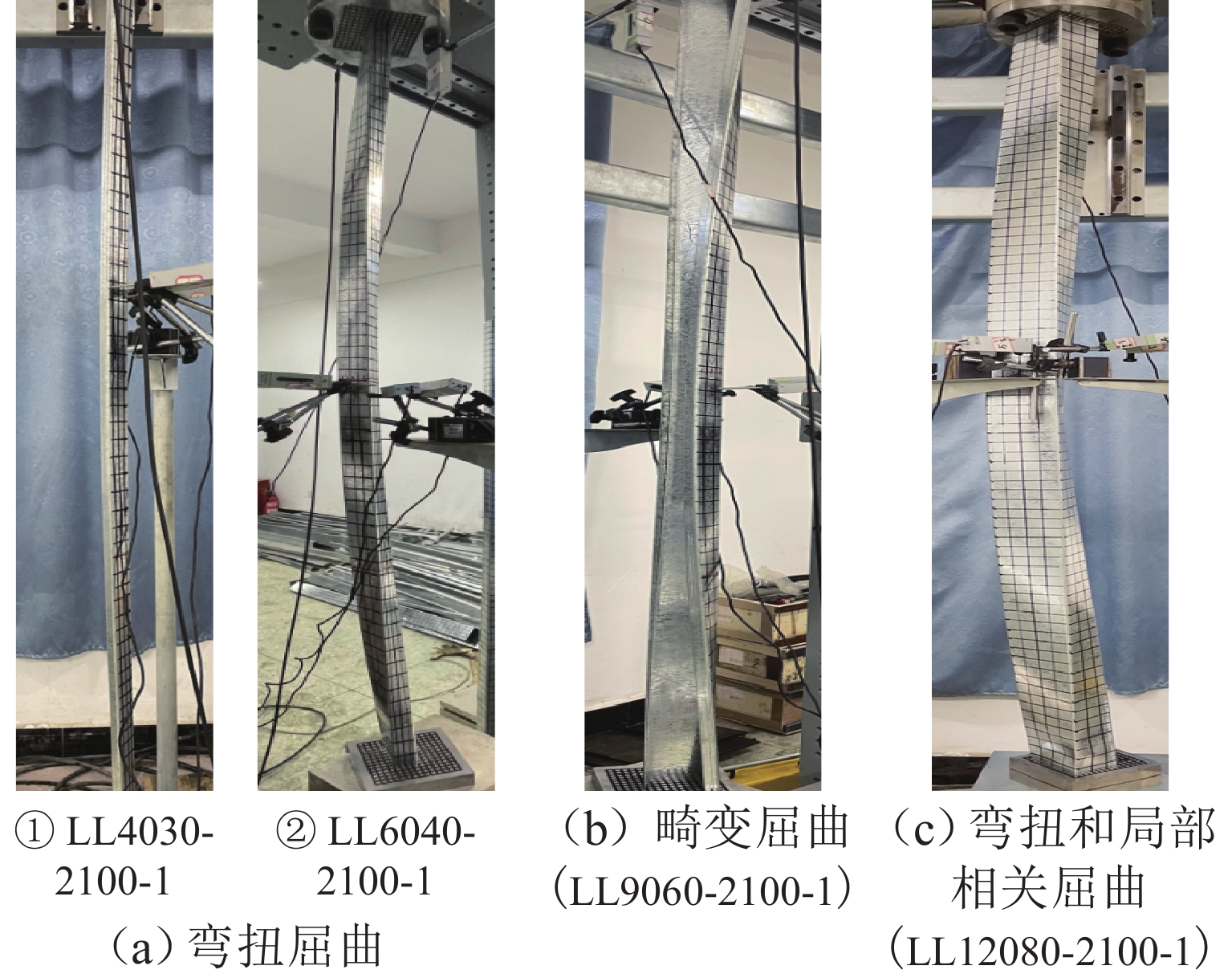
2100 mm角钢柱长度为
2100 mm的不等肢角钢柱屈曲变形如图9所示. 加载初期变形不明显,随着荷载逐渐增大,b2/t=15,20的试件发生明显的弯扭变形,最终表现为弯扭屈曲模式(图9(a)),b2/t=30的试件两肢向内扭转,表现为畸变屈曲(图9(b)),b2/t=40的试件扭转和弯曲变形明显且在构件下端部出现局部屈曲现象,表现为弯扭和局部相关屈曲(图9(c)).由所有试件屈曲模式可知:轴压冷弯薄壁不等肢卷边角钢屈曲模式主要有弯扭屈曲、局部屈曲以及弯扭和局部相关屈曲,个别试件发生了畸变屈曲. 分析发现,长细比大且宽厚比小的试件易发生弯扭屈曲,长细比小且宽厚比大的易发生局部屈曲或者弯扭和局部相关屈曲.
2.2 试验结果
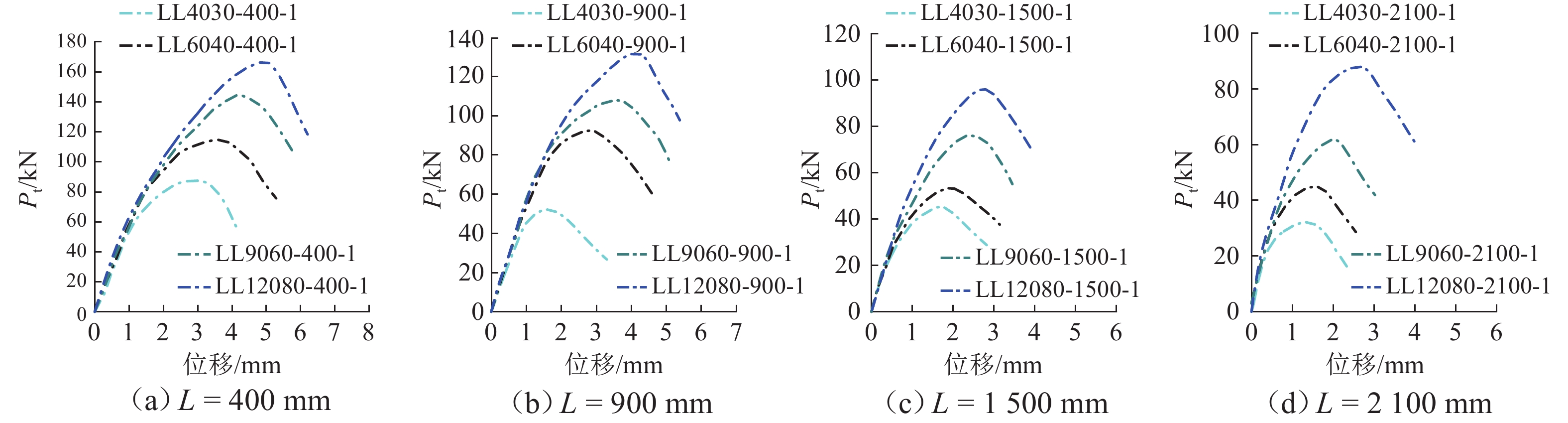
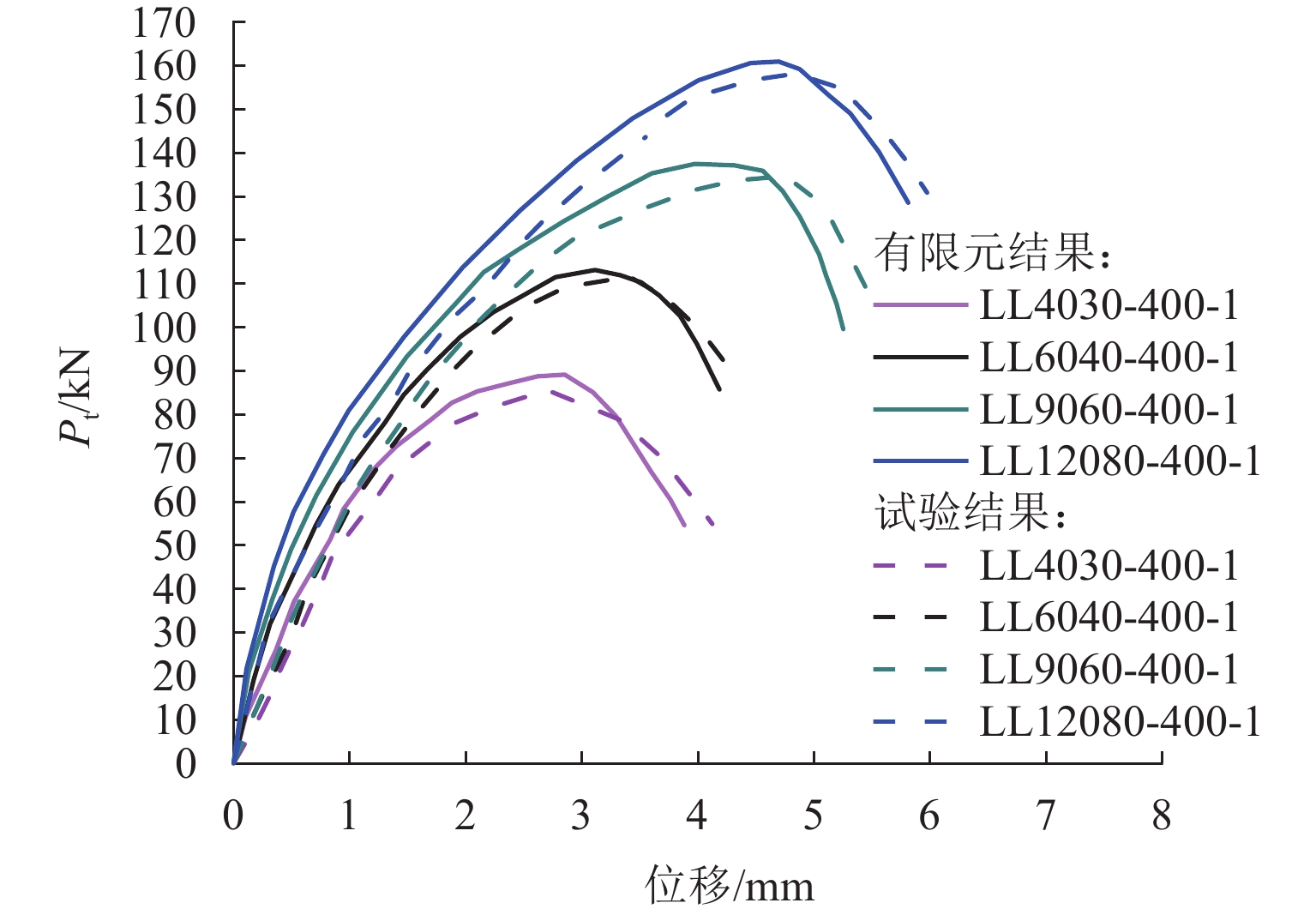
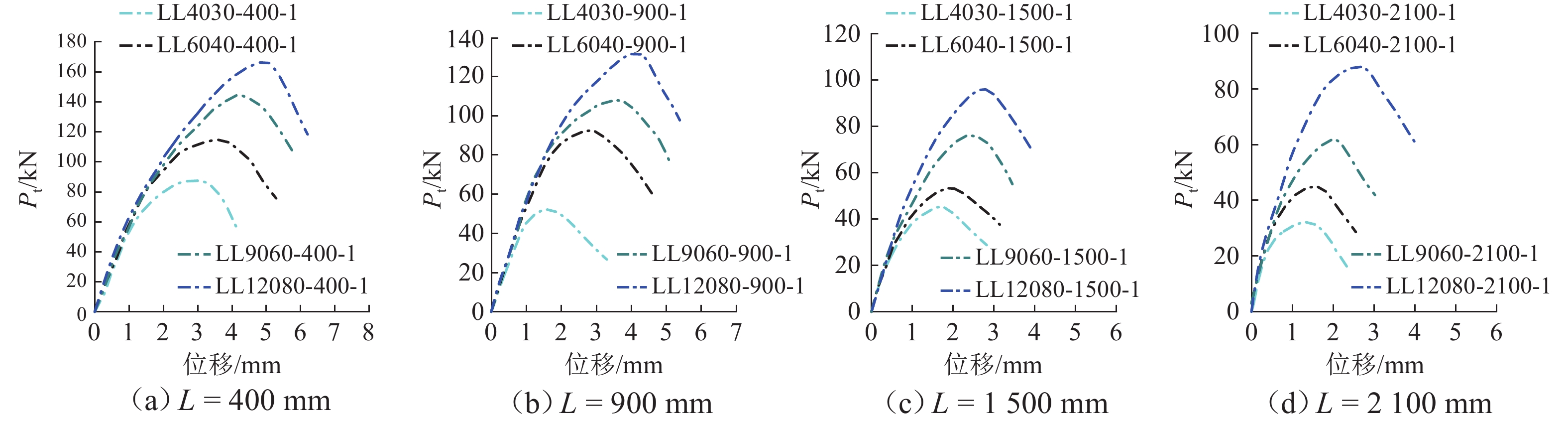
由表3可知,相同试件重复试验的结果非常相近,表明试验结果是可靠的. 相同截面试件的极限承载力随着试件长细比的增加而降低. 相同长度不同截面的试件荷载-位移曲线对比如图10所示:各试件在加载初期处于弹性阶段,荷载变形曲线成线性关系,刚度基本不变,轴向位移随荷载增大而明显增大;试件屈曲后,荷载变形曲线表现为非线性;达到极限荷载后,承载力缓慢降低.
3. 有限元参数化分析
3.1 有限元模型建立
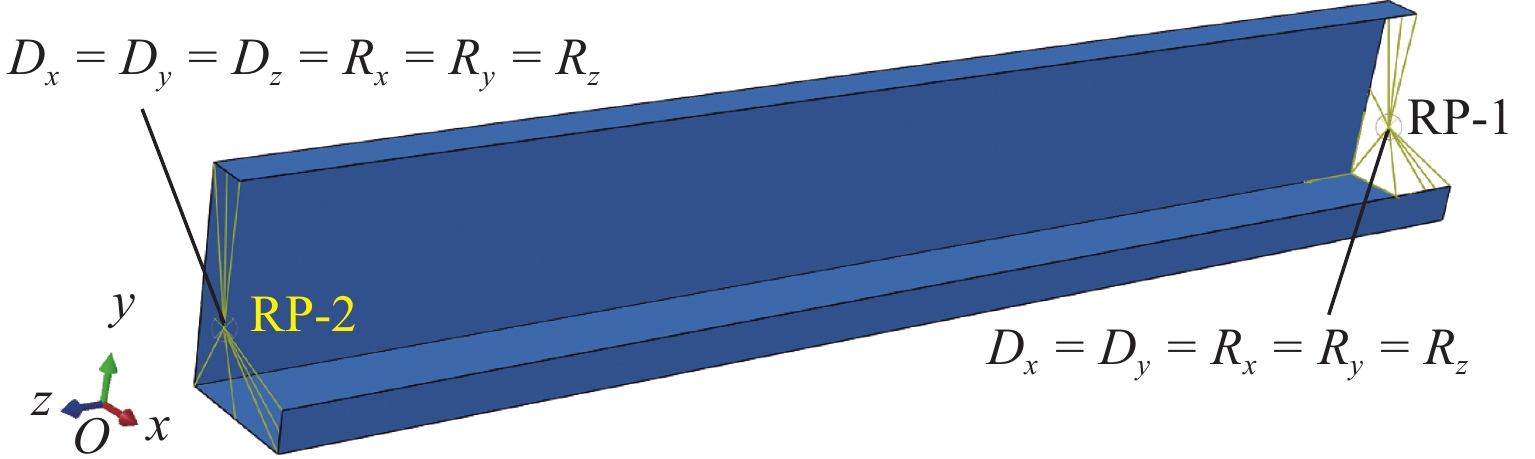
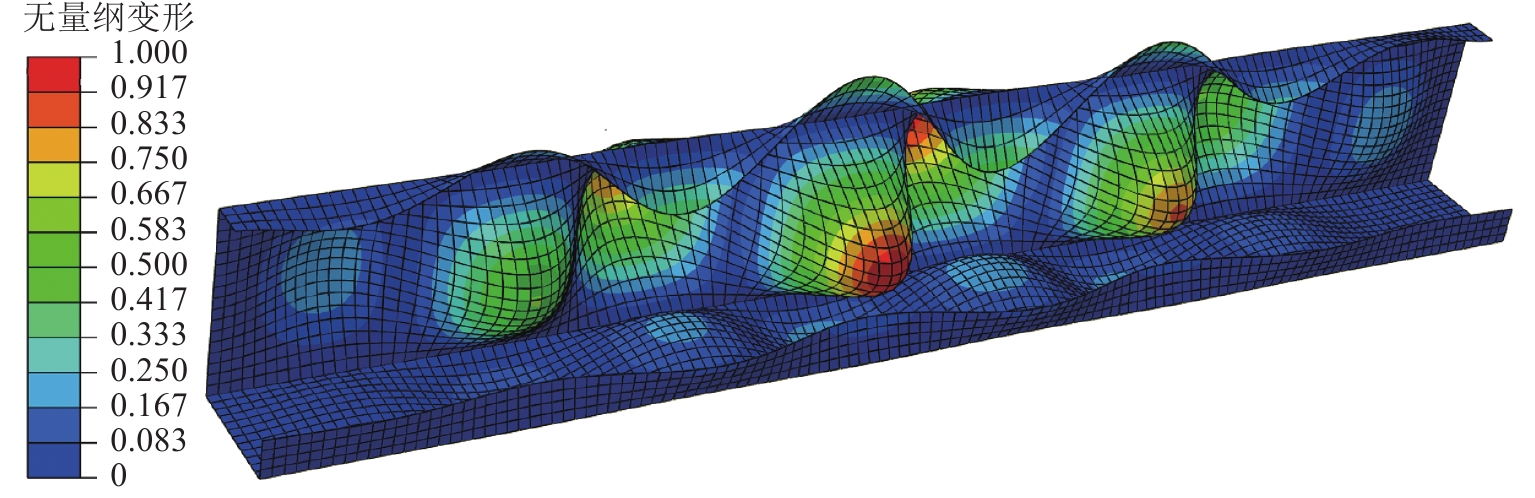
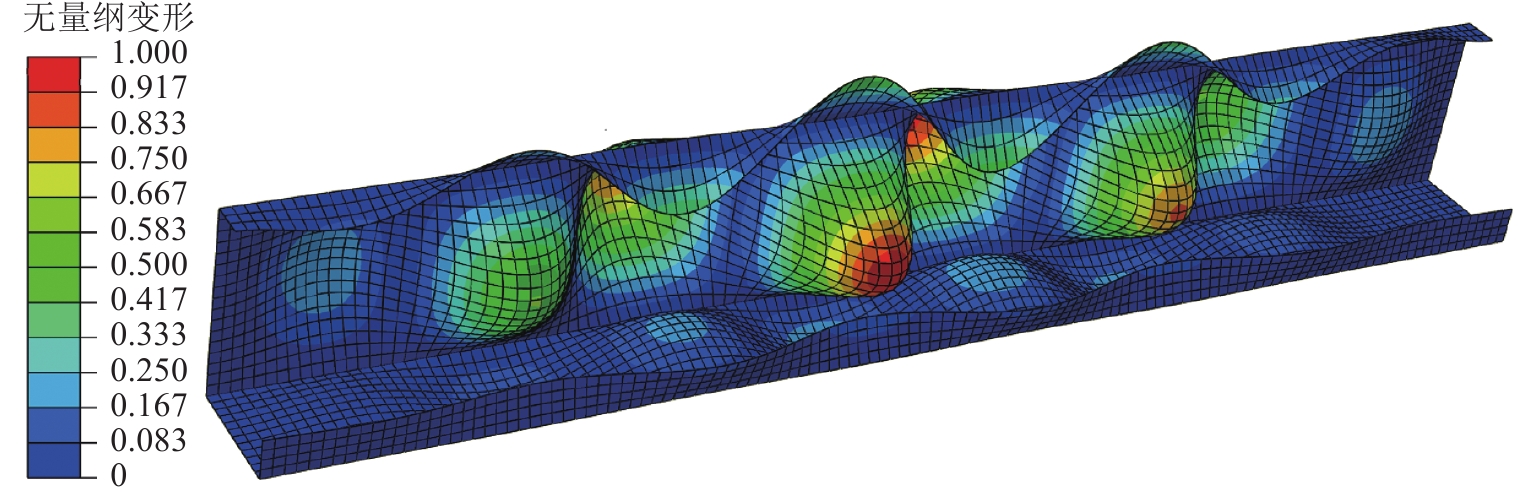
采用ABAQUS有限元软件S4R壳单元和理想弹塑性模型对试件进行数值模拟建模,有限元模型的几何参数及材料特性采用实测值,不考虑残余应力的影响. 建立有限元模型如图11所示,试件上、下端分别耦合于形心RP-1、RP-2位置,上端约束x、y、z方向的平动自由度(Dx、Dy、Dz)以及x、y、z方向的转动自由度(Rx、Ry、Rz),下端约束所有自由度. 有限元分析首先通过弹性特征值屈曲分析获取试件可能出现的第一阶屈曲模态,作为非线性分析的初始几何缺陷形状,图12是试件LL12080-1500-1的一阶弹性屈曲模态,初始缺陷最大值取表3中实测最大值,采用Von-Mises屈服准则,弧长法求解.
3.2 有限元模型验证
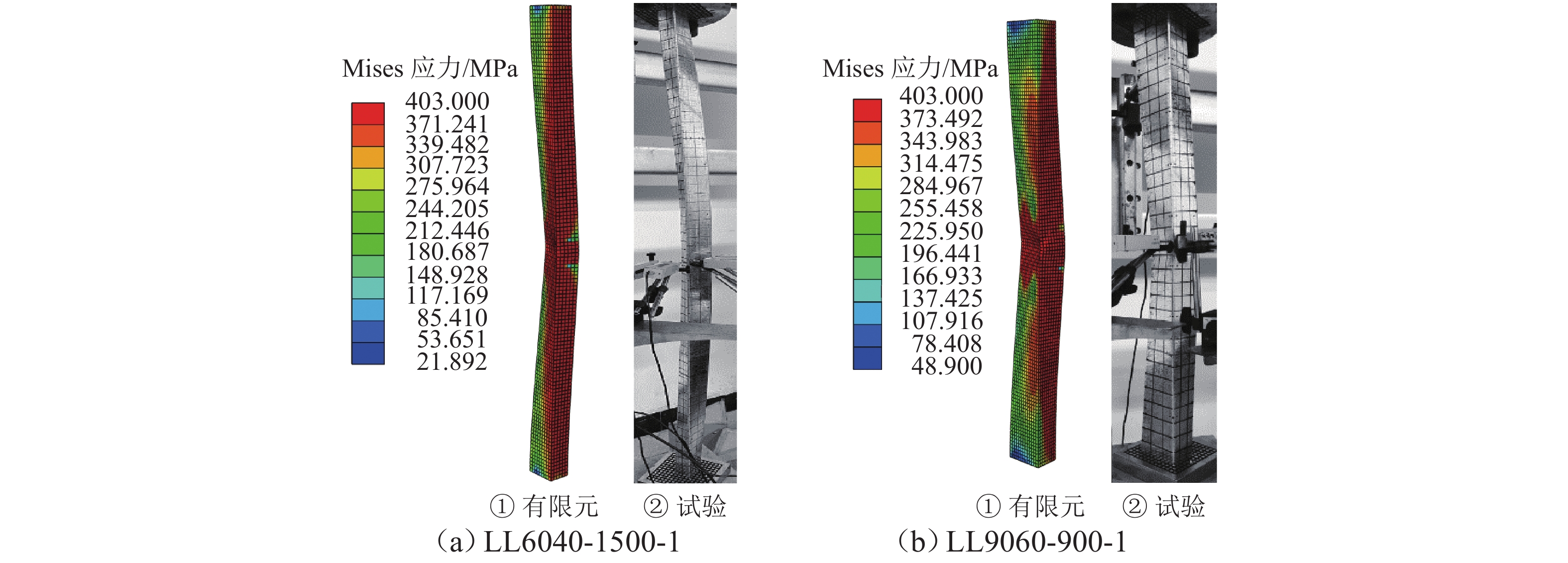
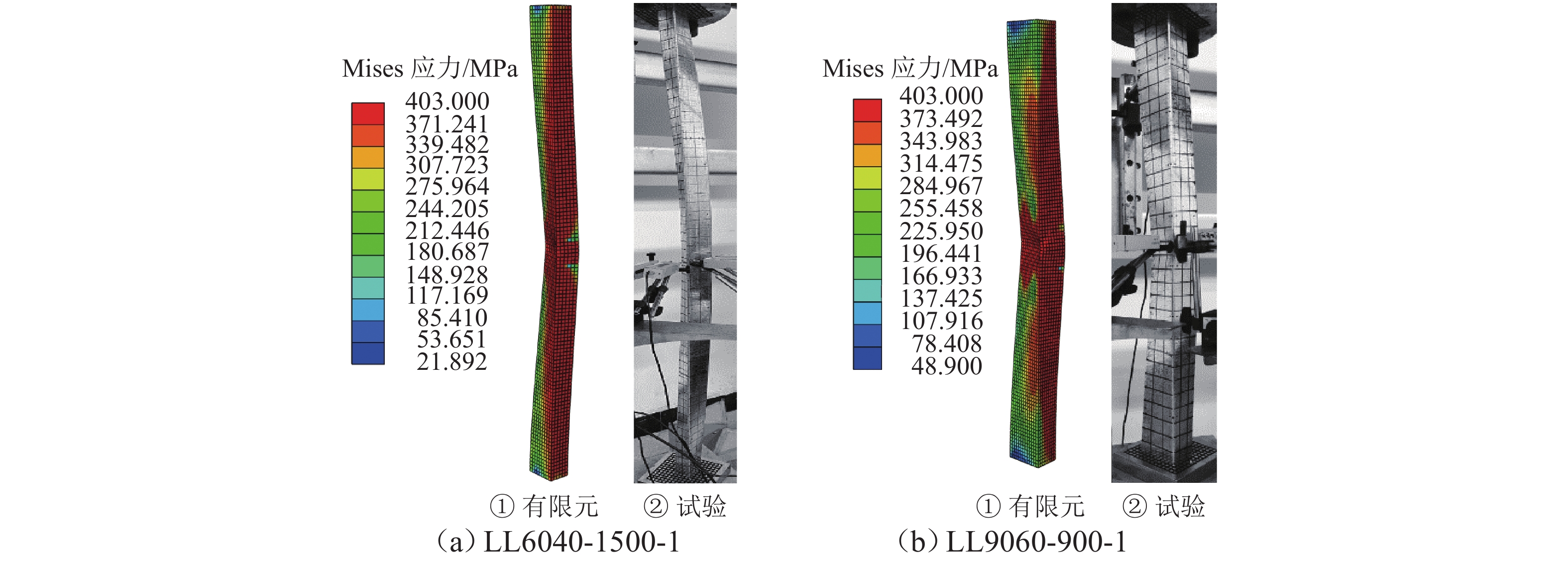
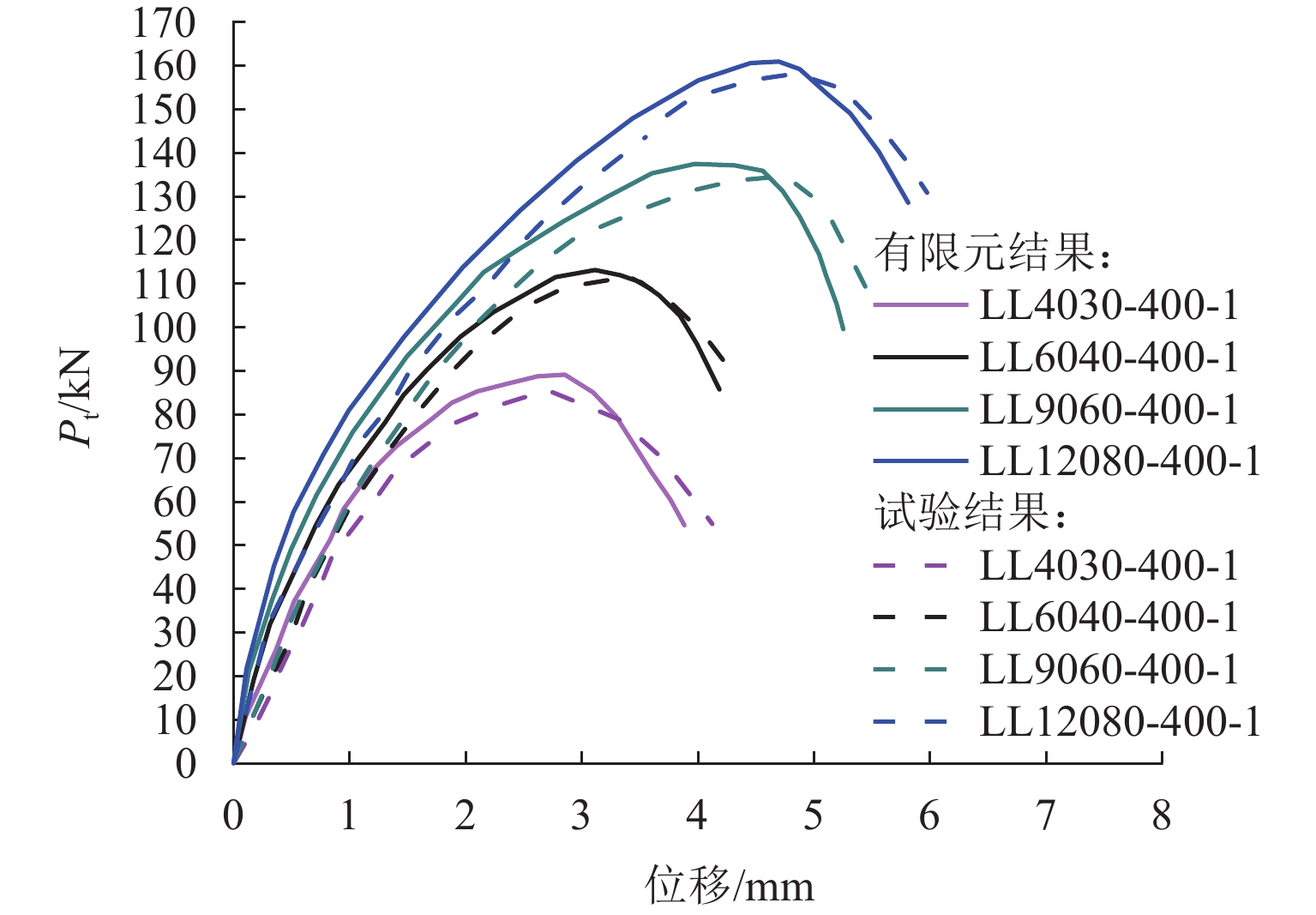
通过ABAQUS有限元软件分析得到所有试件的屈曲模式和极限承载力(表3). 代表性试件的屈曲模式以及荷载-位移曲线对比分别如图13和图14所示. 由表3可知,试件有限元分析屈曲模式与试验结果一致,试验承载力与有限元分析结果比值的平均值和变异系数分别为0.974和
0.0002 . 由图13可知,有限元分析试件LL6040-1500-1和试件LL9060-900-1的弯扭屈曲、弯扭和局部相关屈曲与试验结果一致. 由图14可知,有限元分析试件荷载位移曲线与试验曲线基本一致. 由此表明,本文采用的有限元分析模型是合理可行的.3.3 参数分析
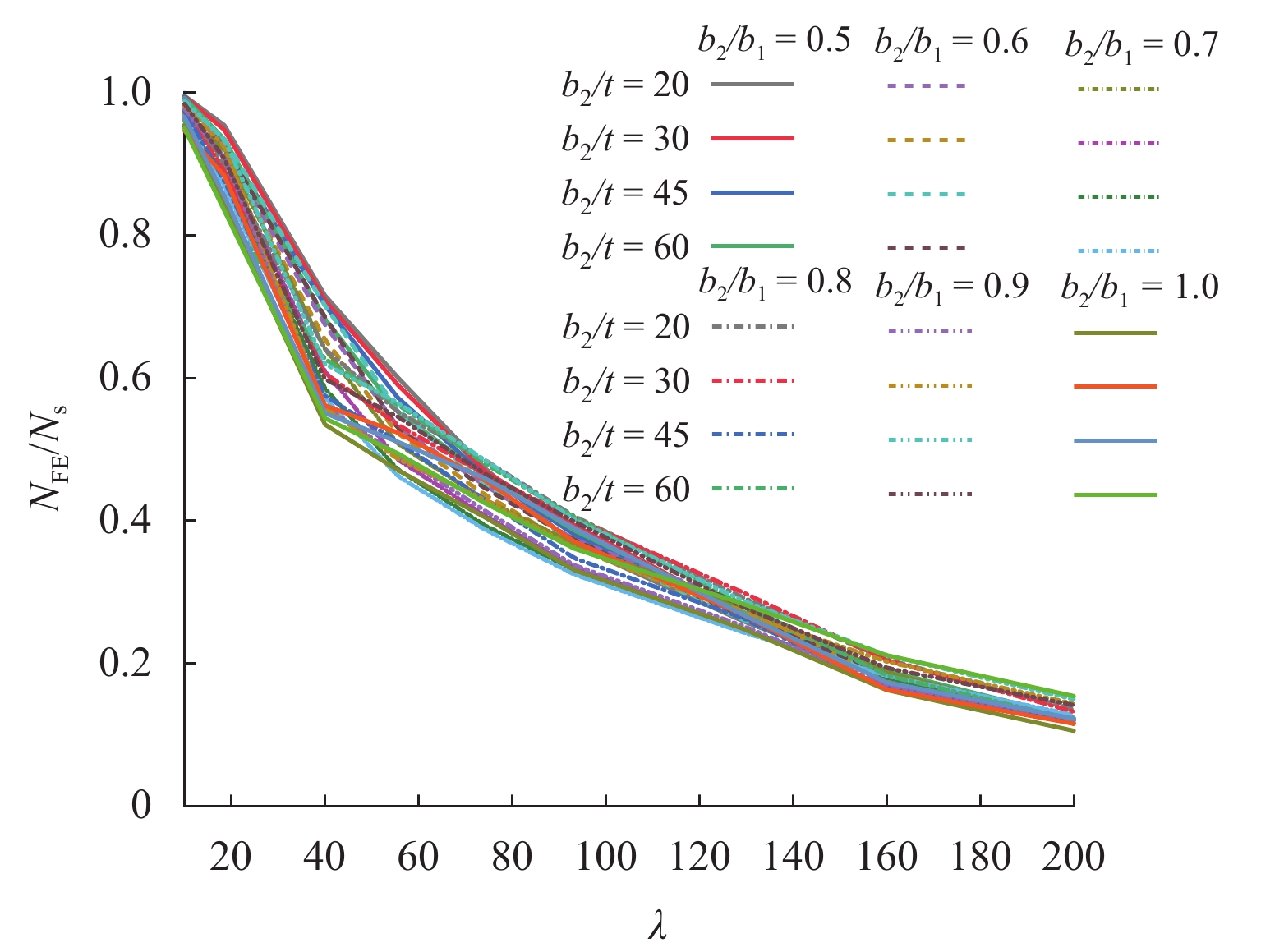
选取不同截面不等肢卷边角钢分析长细比、宽厚比、肢宽比对其承载力的影响. λ=10,20,40,60,80,100,130,160,200;b2/t=20,30,45,60;b2/b1=0.5,0.6,0.7,0.8,0.9,1.0,共216根角钢构件. 得到不同截面和长细比的不等肢卷边角钢极限承载力. 角钢承载力NFE/Ns与长细比之间的关系如图15所示,其中:NFF为有限元分析承载力,Ns为截面屈服承载力. 由图15可知,不等肢卷边角钢极限承载力随构件长细比的增加而降低,当λ<40时影响稍大,长细比对构件极限承载力的影响随构件宽厚比的增大而减弱.
4. 建议计算方法
4.1 现有计算方法
4.1.1 北美规范[4]
北美规范采用直接强度法计算轴心受压冷弯薄壁不等肢卷边角钢构件稳定承载力PD,PD取整体屈曲极限承载力Pne (式(1) )、局部和整体相关屈曲极限承载力Pnl (式(2) )的最小值.
Pne={0.658λ2cPy,λc⩽1.5,0.877λ2cPy,λc>1.5, (1) Pnl={Pne,λl⩽0.776,[1−0.15(PcrlPne)0.4](PcrlPne)0.4Pne,λl>0.776, (2) F3cre(r20−x30−y30)−F2cre[r20(σex+σey+σt)−(σeyx20+σexy20)]+Fcrer20(σexσey+σeyσt+σexσt)−(σexσeyσtr20)=0, (3) 式中:Py为构件屈服承载力,Py=AgFy,Fy为材料屈服强度,Ag为试件的毛截面面积;λc=√Py/Pcre,Pcre为试件弹性整体屈曲临界力,如式(3);λl=√Pne/Pcrl,Pcrl=AgFcrl,为试件弹性局部屈曲临界力,Fcrl为试件弹性局部屈曲临界应力;x0和y0分别为沿x主轴和y主轴从剪心到形心的距离;σex和σey分别为围绕x轴和y轴弹性弯曲屈曲临界应力;r0为弹性扭转屈曲临界应力;σt为r0的回转半径.
4.1.2 Young[5]计算方法
Young考虑角钢构件的整体屈曲后强度,提出建议式:
Pne={0.5λ2cpy,λc⩽1.5,0.5λ2cpy,λc>1.5. (4) 4.1.3 建议方法
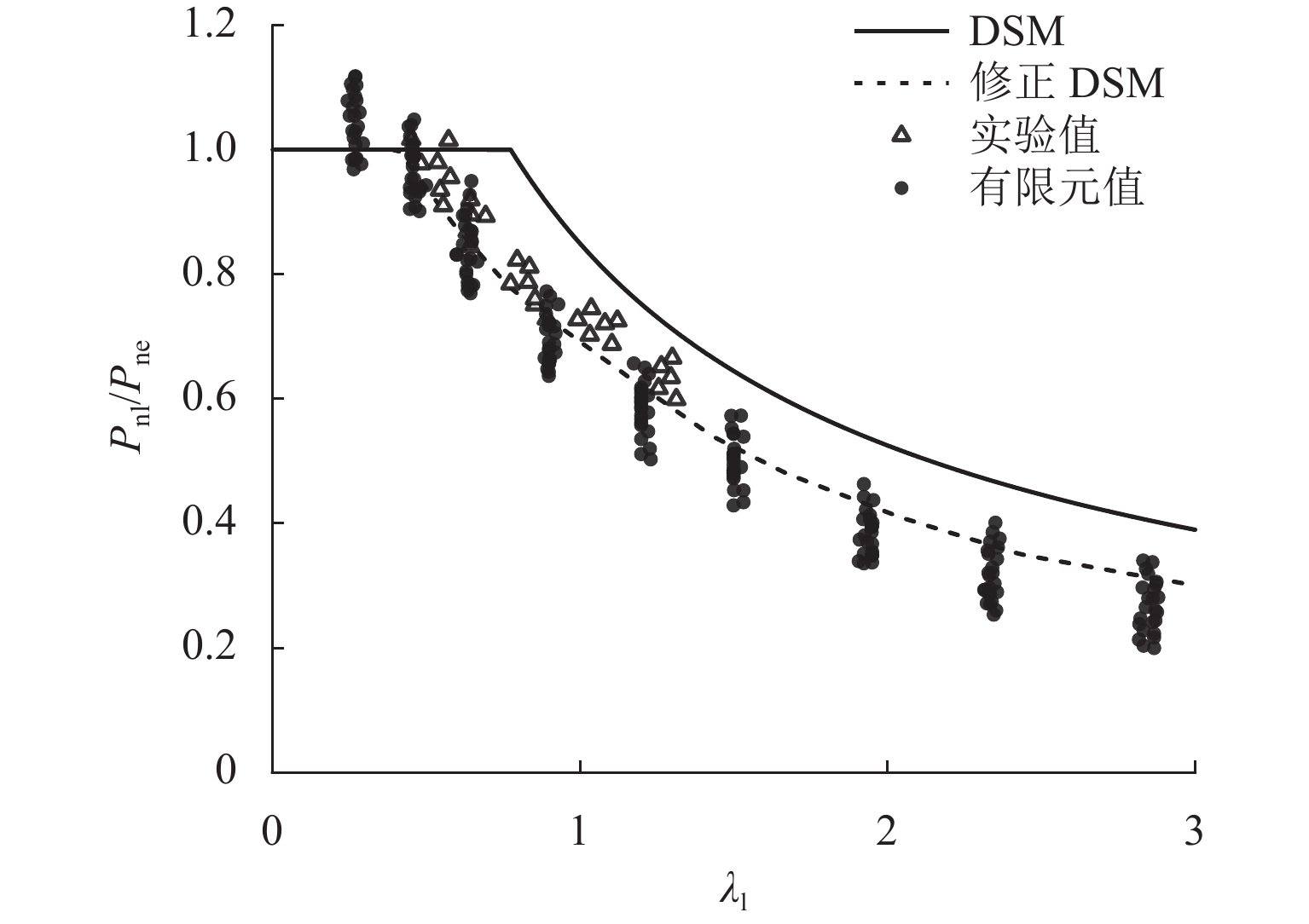
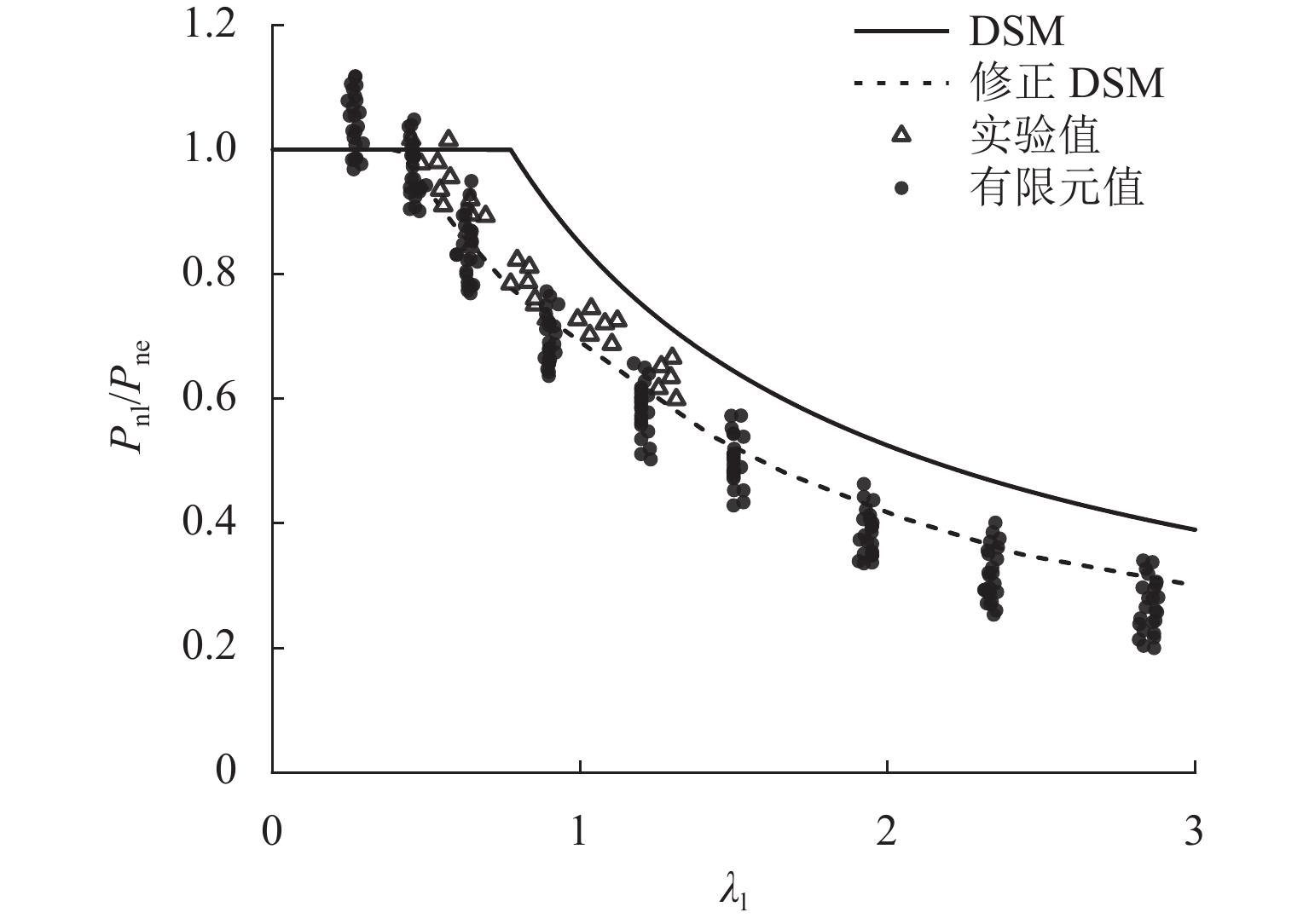
按照北美规范[4]中直接强度法(式(1)~(3))以及Young[5]建议修正式(式(4))计算试件稳定承载力PD和PY,如表3所示. 试件试验极限承载力Pt与北美规范[4]直接强度法计算稳定承载力PD结果之比的均值和变异系数分别为
1.1246 和0.0201 . 试件试验极限承载力Pt和Young[5]建议修正式之比的均值和变异系数分别为1.4760 和0.1395 . 由表3和图16可知,北美规范[4]直接强度法和Young[5]建议方法计算试件稳定承载力偏保守. 图16中,DSM为直接强度法. 为此,基于试验结果及有限元分析结果对直接强度法计算式进行拟合修正,得出Pmne={0.761λ2cPy,λc⩽1.174,0.916λ2cPy,λc>1.174, (5) Pmnl={Pne,λ1⩽0.626,[1−0.191(PcrlPne)0.4](PcrlPne)0.4Pne,λ1>0.626, (6) 式中:Pmne、Pmnl分别为整体屈曲极限承载力和局部和整体相关屈曲极限承载力.
采用修正式(5)、(6)计算得到轴心受压冷弯薄壁不等肢卷边角钢构件稳定承载力PMD,如表3所示,其中,PMD为修正式(5)计算的Pmne和修正计算(式(6))的Pmnl的最小值. 试件试验极限承载力Pt与修正直接强度法PMD计算结果之比的均值和变异系数分别为
1.0318 和0.0074 ,表明修正式是准确的.4.2 建议方法可靠度分析
采用建议的直接强度法(式(5)、 (6))对试验试件、有限元分析构件进行稳定承载力计算,得到采用建议公式计算试验试件以及有限元分析构件的承载力与其之比的均值(变异系数)分别为
1.0318 、0.0075 (0.9769 、0.0109 ). 试件试验极限承载力以及有限元分析的构件承载力与直接强度法曲线及建议公式曲线对比如图16所示. 采用文献[18]一次二阶矩法对冷弯薄壁不等肢卷边角钢设计稳定承载力进行可靠度分析,得到冷弯薄壁型不等肢卷边角钢轴压构件可靠指标如表4所示. 表中:G为恒荷载,L1 和L2分别为办公楼和住宅的活荷载,W为风荷载. 由表4可知,在不同荷载组合下的可靠度指标均大于现行的《冷弯型钢结构技术规范》[16]对LQ550级的冷弯薄壁型钢材的目标可靠指标(3.2). 由此可知,采用修正直接强度法计算此类冷弯薄壁不等肢卷边角钢轴压承载力是安全可靠的.表 4 冷弯薄壁型不等肢卷边角钢轴压构件可靠指标Table 4. Reliability indexes of CFTWS with unequal-leg lipped angles under axial compression类型 ρ2 组合形式 ρ1=0.5 ρ1=1.0 ρ1=2.0 ρ1=3.0 可靠指标平均值 办公楼 0 1.3G + 1.5L1 4.518 4.467 4.442 4.403 4.457 0.5 1.3G + 0.9 × 1.5 (L1 + W) 4.306 4.298 4.253 4.198 4.264 1.0 1.3G + 0.9 × 1.5 (L1 + W) 4.178 4.161 4.102 4.087 4.132 2.0 1.3G + 0.9 × 1.5 (L1 + W) 4.096 4.011 3.918 3.824 3.962 3.0 1.3G + 0.9 × 1.5 (L1 + W) 3.954 3.898 3.837 3.763 3.863 4.0 1.3G + 0.9 × 1.5 (L1 + W) 3.781 3.675 3.621 3.601 3.670 住宅 0 1.3G + 1.5L2 4.924 4.869 4.841 4.799 4.858 0.5 1.3G + 0.9 × 1.5 (L2 + W) 4.693 4.684 4.635 4.575 4.647 1.0 1.3G + 0.9 × 1.5 (L2 + W) 4.554 4.535 4.471 4.454 4.503 2.0 1.3G + 0.9 × 1.5 (L2 + W) 4.464 4.372 4.270 4.168 4.318 3.0 1.3G + 0.9 × 1.5 (L2 + W) 4.309 4.248 4.182 4.101 4.210 4.0 1.3G + 0.9 × 1.5 (L2 + W) 4.121 4.005 3.947 3.925 3.999 注:ρ1 为活荷载标准值与风荷载标准值之和与恒荷载标准值的比值,ρ2 为风荷载标准值与活荷载标准值的比值. 5. 结 论
本文通过对轴心受压冷弯薄壁不等肢卷边角钢进行屈曲承载力试验和有限元分析,得到如下结论:
1) 长细比较大和宽厚比较小的试件易发生弯扭屈曲,长细比较小和宽厚比较大的试件易发生弯扭和局部相关屈曲. 相同长度试件的极限承载力随着宽厚比的增加而提高;相同截面试件的极限承载力随着试件长度的增加而大幅下降;宽厚比越大,构件长度对构件极限承载力的影响越小.
2) 有限元分析结果与试验结果对比表明,建立的ABAQUS有限元分析模型对分析冷弯薄壁不等肢卷边角钢轴压柱屈曲性能是有效的.
3) 有限元参数分析表明,长细比是影响构件极限承载力和屈曲模式的主要因素. 通过增大构件宽厚比和构件肢宽比可提高构件的极限承载力.
4) 北美规范直接强度法建议方法偏保守,基于试验和有限元分析提出的修正直接强度法具有较好的准确性和可靠度.
-
表 1 试件名义几何尺寸
Table 1. Nominal geometric dimension of specimens
试件编号 bl/mm b2/mm al/mm a2/mm t/mm b1/t b2/t LL4030 40 30 15 15 2 20 15 LL6040 60 40 20 20 2 30 20 LL9060 90 60 20 20 2 45 30 LL12080 120 80 24 24 2 60 40 表 2 试件实测几何尺寸
Table 2. Measured geometric dimensions of specimens
试件编号 bl/mm b2/mm al/mm a2/mm t/mm 长度 L/mm 长细比 λ LL4030-400-1 42.13 31.43 15.97 15.54 1.98 400.00 39.19 LL4030-400-2 41.51 31.45 15.56 16.34 1.98 399.27 39.88 LL4030-900-1 42.00 31.61 15.96 14.84 1.99 900.00 87.28 LL4030-900-2 42.25 31.05 15.14 15.79 2.00 900.00 89.45 LL4030-1500-1 41.98 31.48 15.99 15.28 1.97 1498.37 147.14 LL4030-1500-2 41.93 31.42 15.93 15.95 2.00 1499.33 146.65 LL4030-2100-1 42.07 31.32 15.60 15.96 1.98 2101.17 206.51 LL4030-2100-2 42.20 31.59 15.52 15.66 1.98 2101.27 206.93 LL6040-400-1 61.68 41.48 21.63 19.48 1.98 400.00 29.54 LL6040-400-2 61.35 41.52 21.17 21.00 1.97 399.00 29.40 LL6040-900-1 62.05 41.60 21.45 19.37 1.98 900.00 66.46 LL6040-900-2 62.16 41.63 21.54 19.61 2.09 900.00 68.25 LL6040-1500-1 62.10 41.64 21.06 20.48 1.99 1499.43 110.40 LL6040-1500-2 61.80 41.76 21.35 20.98 2.07 1499.27 111.54 LL6040-2100-1 62.24 41.37 21.32 19.86 1.91 2101.10 152.65 LL6040-2100-2 61.44 41.91 20.92 20.47 1.98 2100.00 154.12 LL9060-400-1 91.80 61.41 20.25 20.02 1.97 400.00 22.15 LL9060-400-2 91.92 61.31 20.12 20.50 1.97 399.50 22.16 LL9060-900-1 91.51 61.43 19.90 21.05 1.99 900.00 49.79 LL9060-900-2 91.90 61.89 20.67 20.19 2.00 900.00 49.39 LL9060-1500-1 91.50 62.59 20.51 20.88 2.00 1498.90 81.56 LL9060-1500-2 91.43 62.42 21.08 19.88 1.97 1499.10 81.69 LL9060-2100-1 92.07 61.52 20.99 19.69 1.98 2100.00 115.66 LL9060-2100-2 91.60 61.94 21.83 21.01 2.00 2101.03 113.94 LL12080-400-1 121.53 81.77 24.92 23.98 1.98 400.00 16.95 LL12080-400-2 121.30 81.82 24.19 24.22 1.98 399.27 17.00 LL12080-900-1 122.30 81.71 25.11 24.05 1.97 900.00 38.10 LL12080-900-2 121.87 81.95 23.68 24.90 1.97 900.00 38.23 LL12080-1500-1 121.97 82.20 24.71 24.17 2.00 1498.22 63.34 LL12080-1500-2 122.48 81.87 24.95 24.70 1.97 1499.13 63.33 LL12080-2100-1 122.79 81.89 23.76 24.03 1.98 2101.17 89.38 LL12080-2100-2 121.04 82.24 24.71 24.49 1.97 2100.14 88.61 表 3 试验和有限元分析角钢屈曲模式和承载力对比
Table 3. Comparisons of buckling modes and bearing capacities of steel angles between test and finite element analysis
试件编号 屈曲模式 ΔLmax/
mmΔGmax/
mmPt/kN PFE/kN PD/kN PMD/kN PY/kN Pt/PY Pt/ PFE Pt/ PD Pt/PMD 试验 有限元 一阶模态 LL4030-400-1 FT FT F 0.49 0.28 79.66 83.17 83.77 74.38 72.51 1.0986 0.9578 0.9509 1.0710 LL4030-400-2 FT FT F 0.41 0.34 79.38 82.66 83.67 73.51 73.41 1.0813 0.9603 0.9488 1.0799 LL4030-900-1 FT FT F 1.30 0.83 50.68 53.93 56.47 50.71 27.68 1.8312 0.9397 0.8974 0.9995 LL4030-900-2 FT FT F 1.54 0.77 52.72 54.70 56.90 50.97 27.97 1.8852 0.9638 0.9265 1.0342 LL4030-1500-1 FT FT F 1.30 0.38 40.70 40.93 40.36 42.15 23.01 1.7689 0.9944 1.0085 0.9655 LL4030-1500-2 FT FT F 1.14 0.17 40.98 41.52 41.89 43.75 23.88 1.7159 0.9869 0.9783 0.9366 LL4030-2100-1 FT FT F 1.84 0.08 30.52 31.19 24.48 25.57 13.96 2.1867 0.9786 1.2467 1.1936 LL4030-2100-2 FT FT F 1.10 0.09 29.26 31.35 24.50 25.59 13.97 2.0948 0.9335 1.2343 1.1434 LL6040-400-1 L L L 0.67 0.46 103.70 105.82 102.94 95.81 119.12 0.8706 0.9800 1.0074 1.0824 LL6040-400-2 L L L 0.75 0.31 102.46 105.26 105.33 96.41 121.73 0.8417 0.9734 0.9728 1.0628 LL6040-900-1 FT FT F 1.08 0.63 83.69 85.39 94.17 76.27 52.34 1.5989 0.9800 0.9886 1.0973 LL6040-900-2 FT FT F 1.17 0.74 83.62 84.70 98.45 80.28 54.25 1.5414 0.9872 0.9494 1.0416 LL6040-1500-1 FT FT F 1.15 0.60 59.96 61.98 74.21 61.37 42.31 1.4172 0.9673 0.9480 0.9769 LL6040-1500-2 FT FT F 1.42 0.43 60.82 63.31 78.23 64.30 44.60 1.3638 0.9607 0.9775 0.9459 LL6040-2100-1 FT FT F 1.17 0.24 48.66 50.69 41.59 43.44 23.71 2.0519 0.9600 1.1699 1.1201 LL6040-2100-2 FT FT F 1.42 1.08 49.94 50.96 42.99 44.90 24.51 2.0376 0.9800 1.1617 1.1122 LL9060-400-1 L L L 0.99 0.35 126.76 129.35 104.56 124.03 153.51 0.8257 0.9800 1.2123 1.0220 LL9060-400-2 L L L 0.57 0.57 125.71 129.60 106.03 124.39 155.32 0.8094 0.9700 1.1855 1.0106 LL9060-900-1 FT + L FT + L L 1.12 0.71 94.42 96.00 108.68 101.84 68.10 1.3865 0.9835 0.9688 0.9271 LL9060-900-2 FT + L FT + L L 1.02 0.92 95.60 97.20 107.99 102.07 67.07 1.4253 0.9835 0.9853 0.9366 LL9060-1500-1 FT + L FT + L L 0.79 0.71 77.34 80.36 90.86 94.90 51.80 1.4929 0.9625 0.9512 0.8149 LL9060-1500-2 FT + L FT + L L 0.88 0.83 77.04 78.87 85.54 89.34 48.77 1.5797 0.9768 0.9006 0.8623 LL9060-2100-1 D D F 1.22 0.90 59.95 61.37 48.74 50.90 27.79 2.1574 0.9768 1.2300 1.1776 LL9060-2100-2 D D F 1.08 0.77 61.51 63.42 54.77 57.20 31.22 1.9701 0.9700 1.2232 1.0754 LL12080-400-1 L L L 0.59 0.22 147.54 150.67 117.71 146.76 224.28 0.6578 0.9792 1.2535 1.0053 LL12080-400-2 L L L 0.58 0.14 146.78 150.41 117.29 146.49 223.06 0.6580 0.9759 1.2515 1.0020 LL12080-900-1 FT + L FT + L L 1.20 0.56 136.59 138.37 117.55 118.57 105.70 1.2923 0.9872 1.1620 1.1520 LL12080-900-2 FT + L FT + L L 1.52 1.11 131.36 138.86 118.35 118.60 106.10 1.2380 0.9460 1.1099 1.1075 LL12080-1500-1 FT + L FT + L L 1.20 1.56 97.14 98.99 116.57 104.35 81.31 1.1948 0.9813 0.8334 0.9309 LL12080-1500-2 FT + L FT + L L 1.52 1.11 98.56 98.19 116.67 102.58 83.41 1.1817 1.0038 0.8447 0.9608 LL12080-2100-1 FT + L FT + L L 1.44 1.42 85.43 85.91 73.43 76.89 41.97 2.0354 0.9948 1.2634 1.1110 LL12080-2100-2 FT + L FT + L L 1.45 1.54 85.82 86.48 76.31 80.96 44.19 1.9419 0.9926 1.2246 1.0600 均值 1.4760 0.9741 1.1246 1.0318 方差 0.2060 0.0002 0.0227 0.0077 变异系数 0.1395 0.0002 0.0201 0.0074 表 4 冷弯薄壁型不等肢卷边角钢轴压构件可靠指标
Table 4. Reliability indexes of CFTWS with unequal-leg lipped angles under axial compression
类型 ρ2 组合形式 ρ1=0.5 ρ1=1.0 ρ1=2.0 ρ1=3.0 可靠指标平均值 办公楼 0 1.3G + 1.5L1 4.518 4.467 4.442 4.403 4.457 0.5 1.3G + 0.9 × 1.5 (L1 + W) 4.306 4.298 4.253 4.198 4.264 1.0 1.3G + 0.9 × 1.5 (L1 + W) 4.178 4.161 4.102 4.087 4.132 2.0 1.3G + 0.9 × 1.5 (L1 + W) 4.096 4.011 3.918 3.824 3.962 3.0 1.3G + 0.9 × 1.5 (L1 + W) 3.954 3.898 3.837 3.763 3.863 4.0 1.3G + 0.9 × 1.5 (L1 + W) 3.781 3.675 3.621 3.601 3.670 住宅 0 1.3G + 1.5L2 4.924 4.869 4.841 4.799 4.858 0.5 1.3G + 0.9 × 1.5 (L2 + W) 4.693 4.684 4.635 4.575 4.647 1.0 1.3G + 0.9 × 1.5 (L2 + W) 4.554 4.535 4.471 4.454 4.503 2.0 1.3G + 0.9 × 1.5 (L2 + W) 4.464 4.372 4.270 4.168 4.318 3.0 1.3G + 0.9 × 1.5 (L2 + W) 4.309 4.248 4.182 4.101 4.210 4.0 1.3G + 0.9 × 1.5 (L2 + W) 4.121 4.005 3.947 3.925 3.999 注:ρ1 为活荷载标准值与风荷载标准值之和与恒荷载标准值的比值,ρ2 为风荷载标准值与活荷载标准值的比值. -
[1] 陈明,鲁卫波,武志远,等. 双肢冷弯C型钢蒙古包刚架的平面内稳定性[J]. 西南交通大学学报,2022,57(1):215-222.CHEN Ming, LU Weibo, WU Zhiyuan,et al. Investigation on in-plane stability of double-limb cold-formed C-shaped steel rigid frame of yurt[J]. Journal of Southwest Jiaotong University, 2022, 57(1):215-222. [2] ADLURI S M R, MADUGULA M K S. Flexural buckling of steel angles: experimental investigation[J]. Journal of Structural Engineering, 1996, 122(3): 309-317. doi: 10.1061/(ASCE)0733-9445(1996)122:3(309) [3] DINIS P B, CAMOTIM D, SILVESTRE N. On the mechanics of thin-walled angle column instability[J]. Thin-Walled Structures, 2012, 52: 80-89. doi: 10.1016/j.tws.2011.12.007 [4] American Iron and Steel Institute. North American specification for the design of cold-formed steel structural members: AISI S100-16[S]. Washington D. C.: American Ir-on and Steel Institute, 2016. [5] YOUNG B. Tests and design of fixed-ended cold-formed steel plain angle columns[J]. Journal of Structural Engineering, 2004, 130(12): 1931-1940. doi: 10.1061/(ASCE)0733-9445(2004)130:12(1931) [6] DE BARROS CHODRAUI G M, SHIFFERAW Y, MALITE M, et al, On the stability of cold-formed steel angles undercompression[J]. Rev Esc Minas, 2007, 60(2): 355-363. [7] SILVESTRE N, DINIS P B, CAMOTIM D. Developments on the design of cold-formed steel angles[J]. Journal of Structural Engineering, 2013, 139(5): 680-694. doi: 10.1061/(ASCE)ST.1943-541X.0000670 [8] ELLOBODY E, YOUNG B. Behavior of cold-formed steel plain angle columns[J]. Journal of Structural Engineering, 2005, 131(3): 457-466. doi: 10.1061/(ASCE)0733-9445(2005)131:3(457) [9] YOUNG B, ELLOBODY E. Buckling analysis of cold-formed steel lipped angle columns[J]. Journal of Structural Engineering, 2005, 131(10): 1570-1579. doi: 10.1061/(ASCE)0733-9445(2005)131:10(1570) [10] YOUNG B, CHEN J. Column tests of cold-formed steel non-symmetric lipped angle sections[J]. Journal of Constructional Steel Research, 2008, 64(7): 808-815. [11] SHIFFERAW Y, SCHAFER B W. Cold-formed steel lipped and plain angle columns with fixed ends[J]. Thin-Walled Structures, 2014, 80: 142-152. doi: 10.1016/j.tws.2014.03.001 [12] ZHANG L L, WANG F Y, LIANG Y T, et al. Press-braked S690 high strength steel equal-leg angle and plain channel section stub columns: testing, numerical simulation and design[J]. Engineering Structures, 2019, 201: 109764.1-109764.12. doi: 10.1016/j.engstruct.2019.109764 [13] 姚行友,胡成立,聂泽谕,等. 冷弯不等肢复杂卷边角钢轴压试验及承载力设计方法[J]. 建筑钢结构进展,2024,26(2): 34-44.YAO Xingyou1,HU Chengli,NIE Zeyu,et al. Experiment and design method of cold-formed thin-walled steel unequal-legged complex-lipped angles under axial Compressio[J]. Progress in Steel Building Structures, 2024, 26(9): 34-44. [14] 周宜松. 复杂卷边不等边角形截面冷弯薄壁型钢柱轴压稳定承载力研究[D]. 郑州:郑州大学,2020. [15] 徐步洲. 双卷边不等肢角钢冷弯薄壁型钢柱轴压稳定承载力研究[D]. 西安:西安建筑科技大学,2017. [16] 中华人民共和国建设部,中华人民共和国国家质量监督检验检疫总局. 冷弯薄壁型钢结构技术规范:GB 50018—2002[S]. 北京:中国计划出版社,2002. [17] 国家市场监督管理总局,国家标准化管理委员会. 金属材料拉伸试验第1部分:室温试验方法:GB/T 228.1—2021[S]. 北京:中国标准出版社,2021. [18] 姚行友,李元齐. 考虑畸变屈曲的冷弯薄壁型钢卷边槽形截面构件设计可靠度分析[J]. 建筑钢结构进展,2017,19(6): 65-74.YAO Xingyou, LI Yuanqi. Design Reliability Analysis of Cold-Formed Thin-Walled Steel Members with Lipped Channel Sections Considering Distortional Buckling[J]. Progress in Steel Building Structures, 2024, 19(6): 65-74. -






 下载:
下载:















 下载:
下载: