地铁弓网系统摩擦自激振动研究

冯晓航; 陈光雄; 梅桂明; 董丙杰; 赵鹏鹏; 李先航

doi:10.3969/j.issn.0258-2724.20220873

地铁弓网系统摩擦自激振动研究

doi: 10.3969/j.issn.0258-2724.20220873

1.
西南交通大学机械工程学院，四川成都 610031
2.
西南交通大学轨道交通运载系统全国重点实验室，四川成都 610031

基金项目: 国家自然科学基金项目（52175189）

详细信息

作者简介:
冯晓航（1998—），男，博士研究生，研究方向为轮轨摩擦学，E-mail：fxh1010@my.swjtu.edu.cn

通讯作者:
陈光雄（1962—），男，教授，博士，研究方向为摩擦振动与噪声，E-mail：chen_guangx@163.com

中图分类号: O323
计量
- 文章访问数: 261
- HTML全文浏览量: 122
- PDF下载量: 21
- 被引次数: 0
出版历程
- 收稿日期: 2022-12-19
- 修回日期: 2023-02-21
- 网络出版日期: 2024-06-04

Frictional Self-Excited Vibration of a Metro Pantograph-Catenary System

1.
School of Mechanical Engineering, Southwest Jiaotong University, Chengdu 610031, China
2.
State Key Laboratory of Rail Transit Vehicle System, Southwest Jiaotong University, Chengdu 610031, China

摘要

摘要:
为研究弓网系统摩擦自激振动对碳滑板与接触线之间接触损耗的影响，基于摩擦自激振动理论，建立地铁刚柔过渡段处受电弓-接触网系统有限元模型，并利用复特征值分析方法研究不同弓网参数对该系统摩擦自激振动的影响. 分析结果显示：弓网系统由摩擦自激振动引起接触线波磨产生的主频为399.61 Hz；当摩擦系数大于或等于0.11时，受电弓-接触网系统出现不稳定振动，且摩擦系数越大，该系统出现不稳定振动的趋势越强；法向接触力、碳滑板与接触线的接触位置以及弓头弹簧刚度对弓网系统摩擦自激振动的产生有很大影响；摩擦系数低于0.11并且选择合适的法向接触力或调整弓头弹簧刚度可以抑制甚至消除弓网系统的摩擦自激振动，进而减少弓/网间摩擦引起的接触损耗.
- 弓网系统 /
- 刚柔过渡 /
- 摩擦自激振动 /
- 复特征值分析 /
- 数值仿真
Abstract:
In order to study the influence of frictional self-excited vibration of a pantograph-catenary system on the contact loss between the carbon strip and contact wire, a finite element model of the pantograph-catenary system at the rigid and flexible transition section of a metro was established based on the theory of frictional self-excited vibration. The complex eigenvalue analysis method was used to study the influence of different pantograph-catenary parameters on the frictional self-excited vibration of the system. The analysis results show that the main frequency of contact line corrugation caused by frictional self-excited vibration of the pantograph-catenary system is 399.61 Hz. When the friction coefficient is greater than or equal to 0.11, the pantograph-catenary system has unstable vibration, and with the increase in the friction coefficient, the unstable vibration tends to be stronger. The normal contact force, the contact position between the carbon strip and the contact wire, and the stiffness of the pantograph bow spring have great influences on the occurrence of the frictional self-excited vibration of the pantograph-catenary system. When the friction coefficient is less than 0.11, selecting the appropriate normal contact force or adjusting the stiffness of the pantograph bow spring can restrain or even eliminate the frictional self-excited vibration of the pantograph-catenary system and then reduce the contact loss caused by the friction between the pantograph and catenary.
- pantograph-catenary system /
- rigid and flexible transition /
- frictional self-excited vibration /
- complex eigenvalue analysis /
- numerical simulation

HTML全文

近年来，我国城市轨道交通飞速发展，地铁已经成为人们出行的必要交通工具. 目前某些城市由于环境因素的限制，在有隧道的线路上铺设接触网时一般会使用刚性与柔性接触网相结合的形式，此时便会伴随着刚柔过渡结构的出现^[1-3]. 列车一般通过受电弓从接触网上收集电力，为地铁列车的正常运行提供能量. 但弓网系统中碳滑板与接触线之间的接触磨损会产生电弧放电，进一步加重了碳滑板的磨损^[4-7]，从而导致列车的受流质量严重降低. 而当刚柔过渡结构存在时，弓网系统的接触刚度就会发生变化，可能会进一步降低弓网的稳定接触和受流质量. 因此，提高列车的受流质量，缓解或者抑制碳滑板与接触线之间的接触损耗是很有必要的.

大多数学者认为接触损耗是接触线不平顺和车体振动导致弓网系统振动引起的. Mandai等^[8]认为，刚性接触网接触线的刚度不均匀性会严重影响弓网系统的受流质量，并设计了一种可以有效降低接触损耗的磨床. Wu等^[9]建立弓网系统周期激励的单自由度模型，研究弓网系统的动力特性，并证明了接触网的动刚度随列车速度的变化而变化. Chen等^[10]研究了运行参数对弓网系统性能的影响，并发现接触线某些特定波长的不平顺性对弓网系统性能的影响要远大于其他波长. Masatoshi等^[11]认为接触线异常磨损主要是波动磨损，并且在光滑的接触线表面上接触损耗最小，不会产生波动磨损. 钱世勇等^[12]发现，弓头的剧烈振动会导致弓网接触压力动态范围变大,加剧刚性接触网的波形磨耗，降低接触线使用寿命. 谭冬华^[13]对刚性接触网在运营过程中出现弓网异常磨耗的现象进行了分析，认为接触线和碳滑板的接触磨耗是刚性接触网相对于受电弓中心偏移导致的. 裴志禹^[14]通过建立弓网系统耦合模型发现，在刚柔过渡结构处，接触刚度减小时，接触力的波动也会减小，在一定程度上有可能改善弓网系统的动态特性.

我国近年来出现的刚性接触网系统弓网严重磨损的问题没有得到根本解决，有学者认为是由于弓网系统的摩擦自激振动引起的，但这方面的研究极少. Qian等^[15]利用复特征值法研究高铁柔性接触网与受电弓之间的摩擦自激振动现象，证明了弓网系统摩擦特性对弓网接触状态有很大的影响. 目前也有很多学者利用复特征值分析方法对轮轨系统中的摩擦自激振动现象进行研究，并取得了丰硕的成果^[16-19]. 本文基于摩擦自激振动理论，建立包含刚性接触网、柔性接触网和刚柔过渡结构的弓网系统有限元模型，利用复特征值分析方法研究了不同弓网参数对地铁弓网系统摩擦自激振动的影响.

1. 弓网系统有限元模型和理论方法

1.1 接触网模型

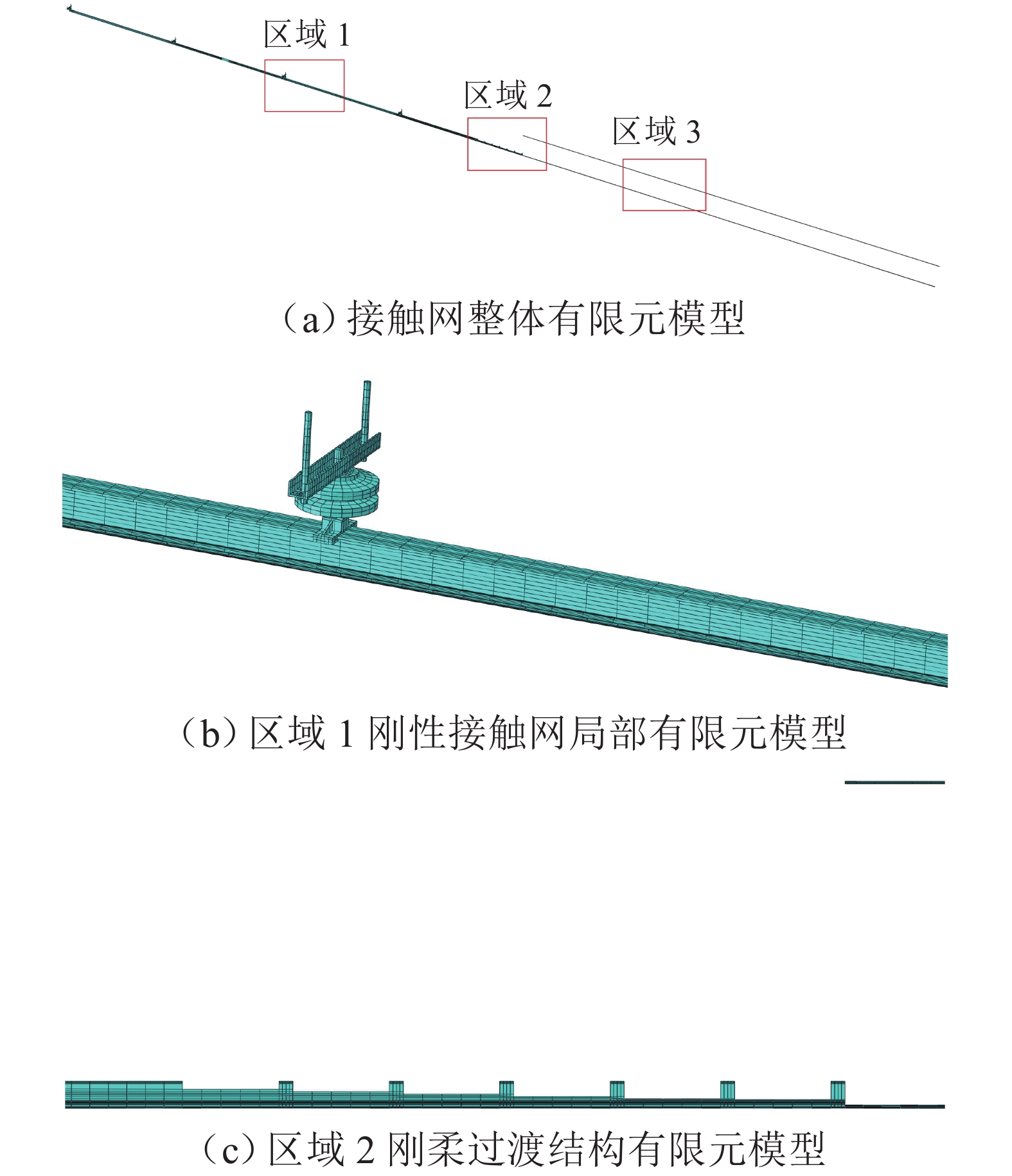
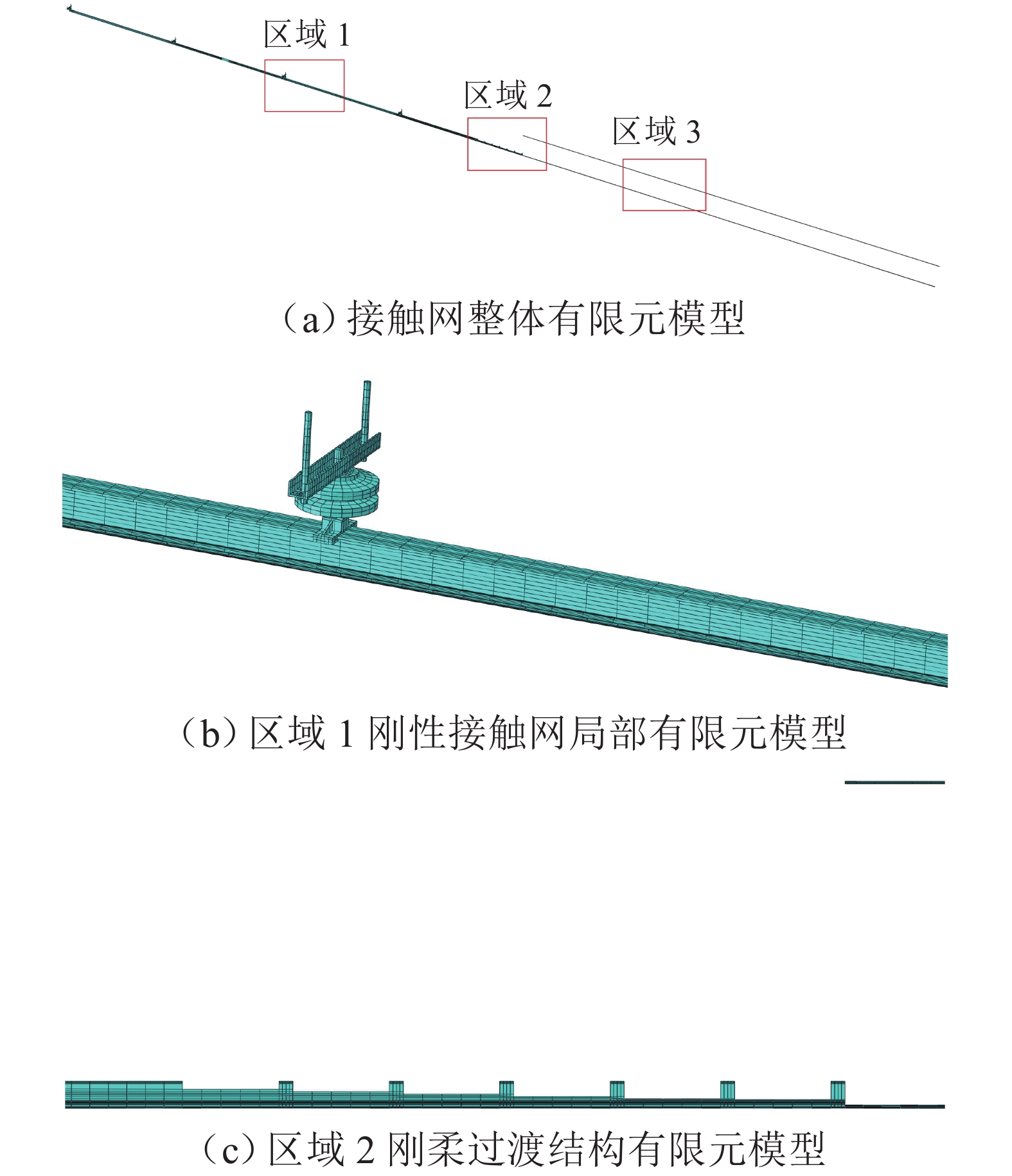
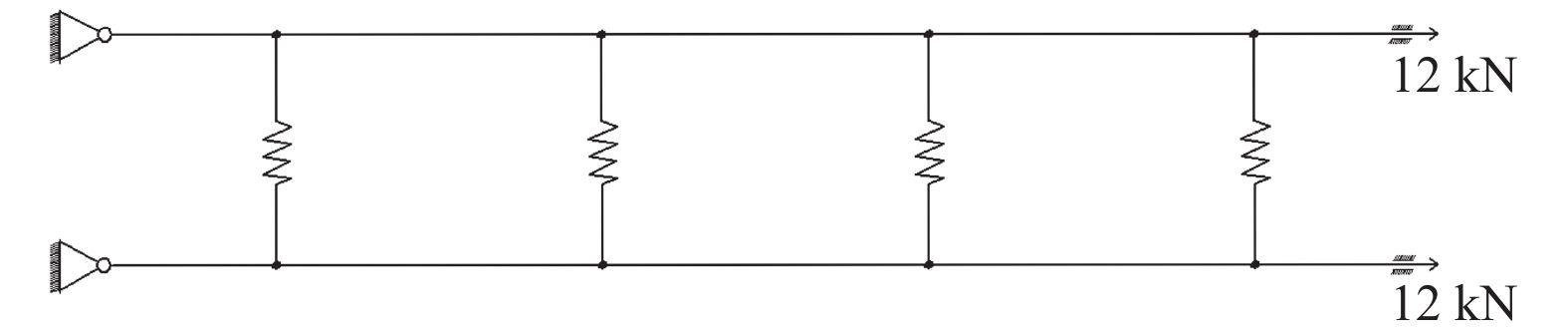
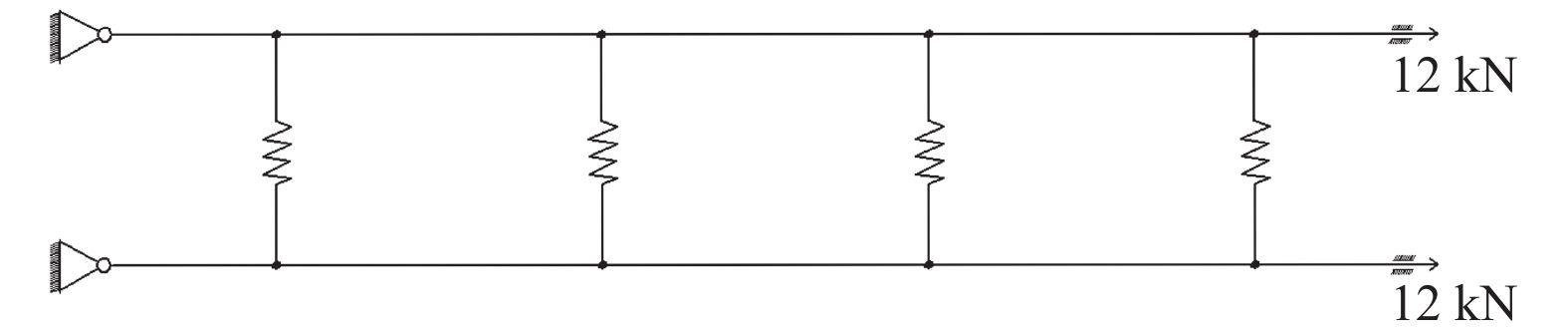
接触网局部有限元模型如图1所示，其主要由区域1、2和3，即刚性接触网、刚柔过渡段以及柔性接触网3部分组成. 整个接触网模型均采用六面体实体单元（C3D8I），网格数量为71684个. 图1（b）为刚性接触网局部有限元模型，由接触线、汇流排以及悬挂机构组成，悬挂机构为4个，跨距为8 m，模型主要参数^[20-21]如表1所示. 图1（c）为刚柔过渡结构有限元模型，其是由刚度逐渐减小的汇流排与柔性接触线连接起来的贯通式刚柔过渡段. 图2为柔性接触网模型，由接触线、承力索以及吊弦组成，其中吊弦用弹簧单元代替，模型主要参数^[22]见表2，其中单跨吊弦数为3根，张力为12 kN，吊弦间距为4、8、8、4 m.

图 1 接触网有限元模型

Figure 1. Finite element model of catenary

下载: 全尺寸图片幻灯片

表 1 刚性接触网模型参数

Table 1. Rigid catenary model parameters

部件	材料	密度/ （× 10³ kg•m⁻³）	弹性模量/ GPa	泊松比
汇流排	6101B	2.71	69	0.33
接触线	CTHA120	8.92	130	0.30
定位线夹	铝合金	2.70	70	0.34
绝缘子	陶瓷	3.80	340	0.22

下载: 导出CSV

| 显示表格

图 2 柔性接触网模型

Figure 2. Flexible catenary model

下载: 全尺寸图片幻灯片

表 2 柔性接触网模型参数

Table 2. Flexible catenary model parameters

部件	材料	密度/ （× 10³ kg•m⁻³）	弹性模量/ GPa	泊松比
承力索	JT150	9.20	105	0.30
接触线	CTHA120	8.92	130	0.30

下载: 导出CSV

| 显示表格

1.2 受电弓模型

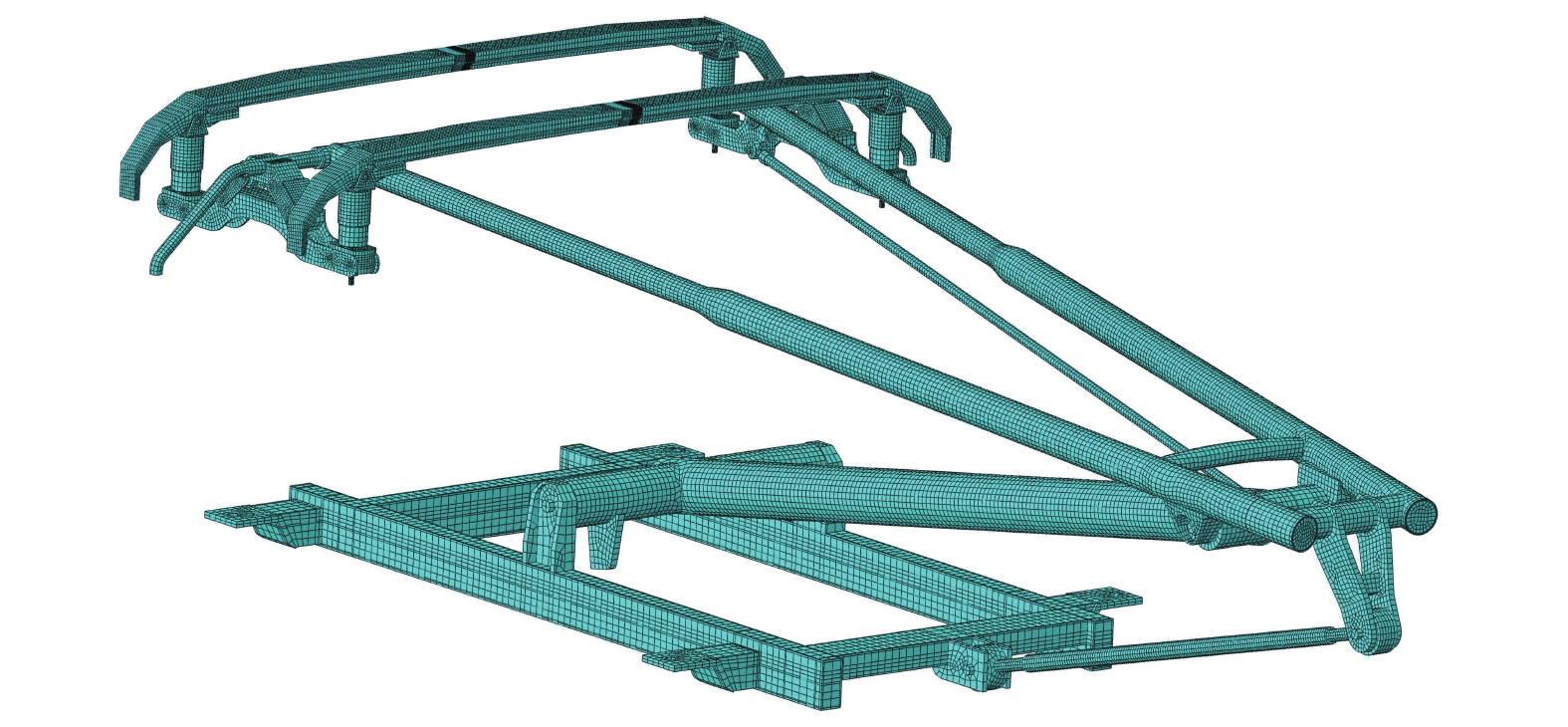
本文地铁受电弓型号为TSG22型，其有限元模型如图3所示，主要由碳滑板、弓头支架、上框架、下臂杆、平衡杆和底座等部件组成. 模型底座固定，转动部分均通过铰链进行连接，其平行四边形结构可以确保施加在下臂杆上的力矩产生固定的静态升弓力. 整个受电弓模型均采用六面体实体单元（C3D8I），网格数量为107073个，模型主要参数^[15,20]见表3.

图 3 受电弓有限元模型

Figure 3. Finite element model of pantograph

下载: 全尺寸图片幻灯片

表 3 受电弓各部件材料

Table 3. Material properties of pantograph components

部件	材料	密度/ （ × 10³ kg•m^–3）	弹性模量/ GPa	泊松比
平衡杆	碳纤维	1.9	231	0.23
碳滑板	铜、碳	2.4	12.6	0.43
下臂杆	铝合金	2.8	72	0.33
弓头支架	钛合金	4.5	117	0.34
其他部件	Q235	7.8	210	0.30

下载: 导出CSV

| 显示表格

1.3 弓网系统耦合模型

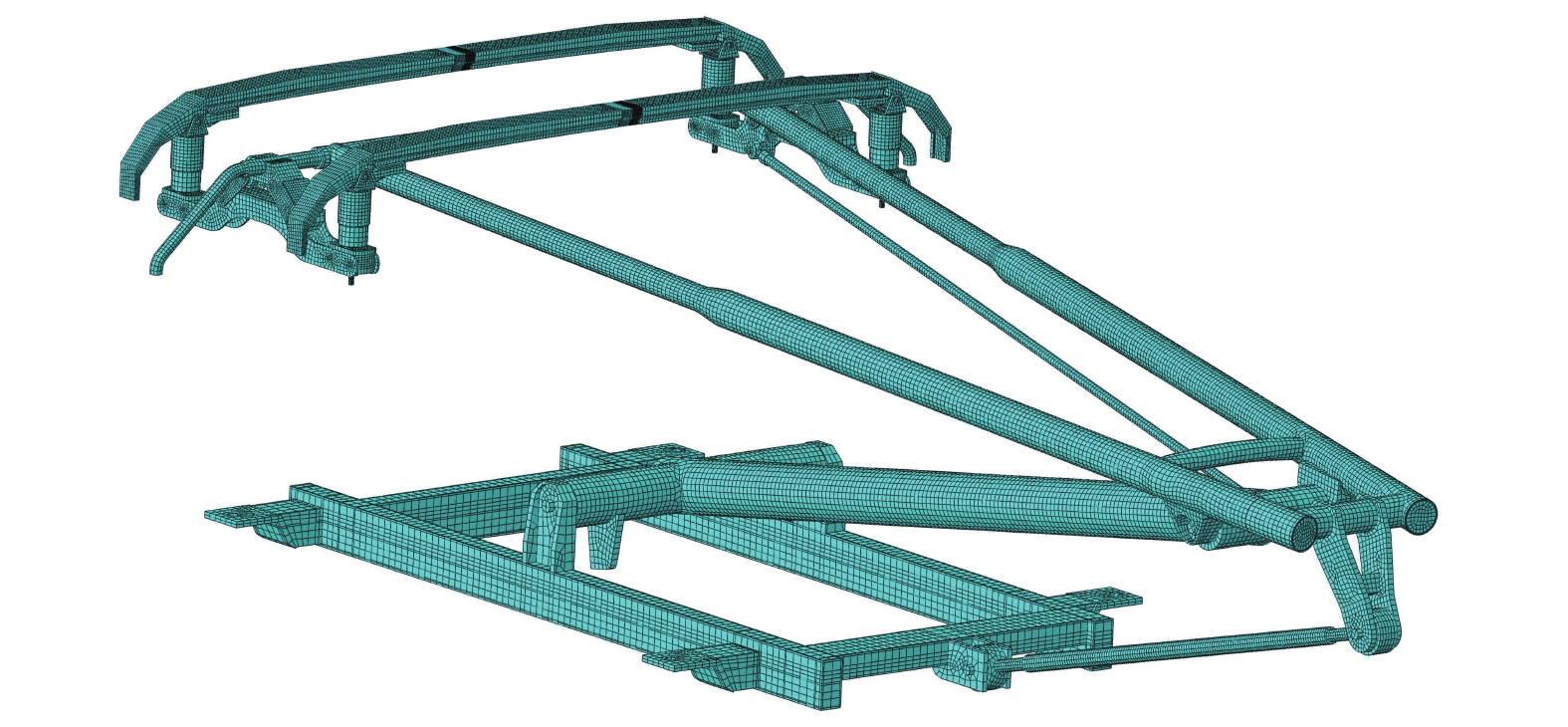
将接触网模型和受电弓模型结合起来，得到弓网系统耦合模型，见图4. 整个有限元模型共有178757个六面体实体单元（C3D8I）. 此模型中碳滑板与接触线之间的接触是一个很重要的部分，本文主要研究弓网系统存在摩擦时的自激振动，模型中存在摩擦时，受电弓和接触网的相互作用是非常复杂的，其复杂性在于碳滑板与接触线之间的摩擦耦合. 本文以Yuan^[23]的方法来添加碳滑板和接触线之间的摩擦耦合. 当不考虑外力和摩擦力时，建立如式（1）的系统运动微分方程.

图 4 弓网系统有限元模型

Figure 4. Finite element model of pantograph-catenary system

下载: 全尺寸图片幻灯片

$\boldsymbol{M}\ddot{\boldsymbol{x}} + \boldsymbol{C}\dot{\boldsymbol{x}} + \boldsymbol{K}\boldsymbol{x}={\boldsymbol{0}} \text{，}$

(1)

式中：M为质量矩阵，C为阻尼矩阵，K为刚度矩阵，x为位移矢量.

M、C、K均为对称矩阵，此时式（1）特征方程不会出现实部大于0的特征值，即系统是稳定的.

加入摩擦耦合后，系统动力学方程转变成

${\boldsymbol{M}}_{\rm{r}}\ddot{\boldsymbol{x}} + {\boldsymbol{C}}_{\rm{r}}\dot{\boldsymbol{x}} + {\boldsymbol{K}}_{\rm{r}}\boldsymbol{x}={\boldsymbol{0}} \text{，}$

(2)

式中：M_r为化简后质量矩阵，C_r为化简后阻尼矩阵，K_r为化简后系统刚度矩阵.

对式（2）进行求解得

${\boldsymbol{x}}\left(t\right)={\sum }_{k=1}^{n}{\boldsymbol{\varphi }}_{{k}}{{\mathrm{exp}}}\left(\left({\beta }_{k} + {w}_{k}{\rm{j}}\right)t\right) \text{，}$

(3)

式中： ${\beta }_{k} + {w}_{k}{\rm{j}}$ 为系统特征值， ${\beta }_{k}$ 为特征值实部， ${w}_{k}$ 为特征值虚部； ${\boldsymbol{\varphi }}_{{k}}$ 为特征向量； $t$ 为时间.

由于M_r、C_r、K_r均为非对称矩阵，因此 ${\beta }_{k}$ > 0， ${\boldsymbol{x}}\left(t\right)$ 随 $t$ 呈指数增大，表明弓网系统的振动将变得不稳定. 通常使用等效阻尼比ζ来评估弓网系统的稳定性，如式（4）.

${\zeta }_{}=-\frac{2{\beta }_{k}}{\left|{w }_{k}\right|} .$

(4)

当ζ > −0.001时，由于系统阻尼的影响，此振动不会一直发展下去，可忽略此时的振动频率；当ζ < −0.001时，ζ越小，弓网系统产生摩擦自激振动的可能性越大，越容易出现接触线波磨.

2. 计算结果分析

2.1 模型验证

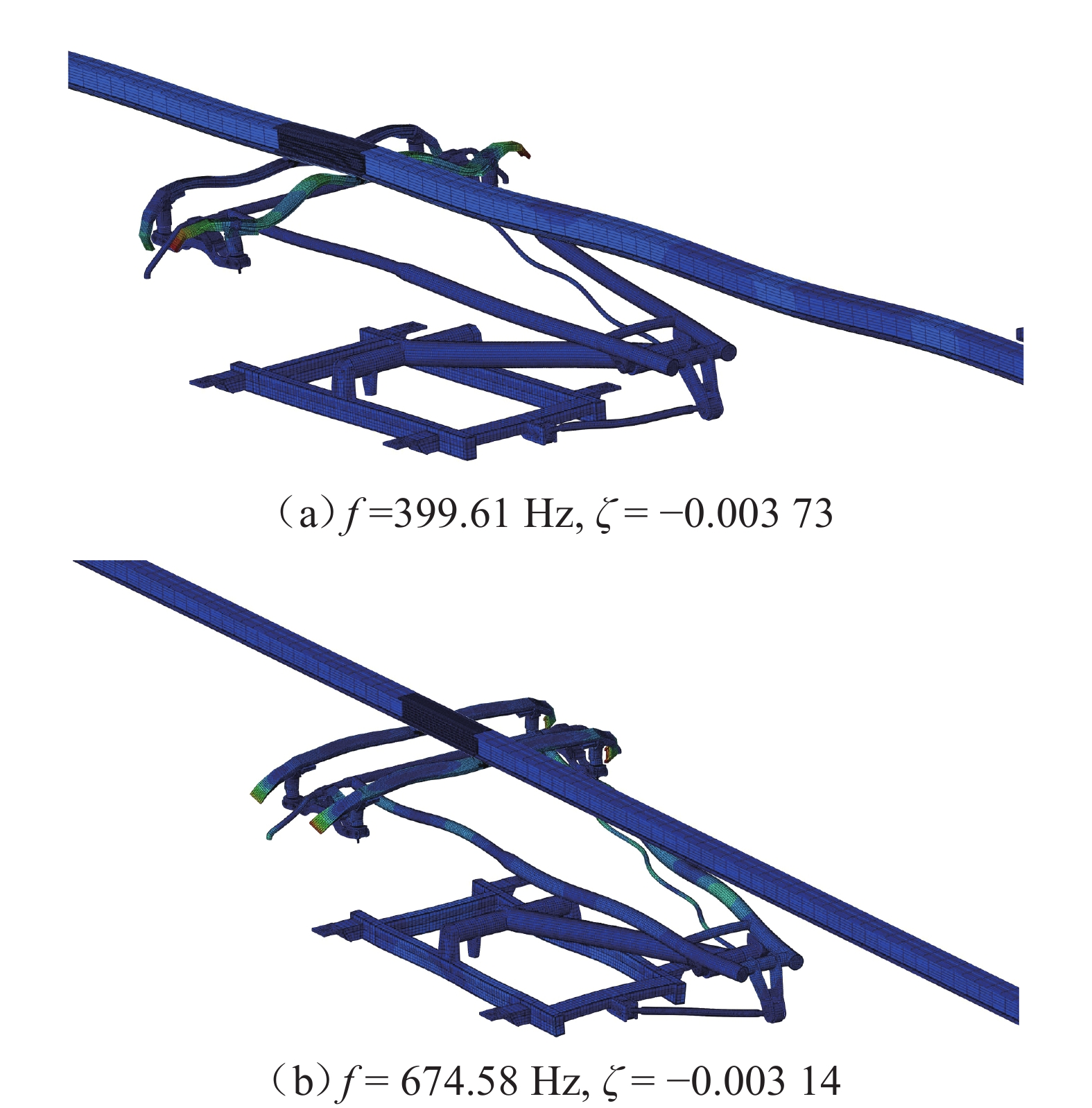
在摩擦系数μ = 0.3，法向接触力F = 120 N，刚性接触网位于碳滑板中间位置，弓头弹簧刚度K = 3600 N/m，频率的求解范围为0～1200 Hz时，弓网系统会在399.61 Hz和674.58 Hz处发生不稳定振动现象，振动模态如图5所示. 从图5（a）可以看出，频率为399.61 Hz时，弓网系统的振动主要集中在碳滑板和接触网处. 从图5（b）可以看出，频率f = 674.58 Hz时，弓网系统的振动主要发生在碳滑板、上框架和平衡杆处. 结合等效阻尼比的数值与弓网系统在各频率下的振动模态可以推测出频率ƒ=399.61 Hz更容易使弓网系统产生摩擦自激振动，进而导致波磨出现.

图 5 刚性弓网系统不稳定振动模态

Figure 5. Unstable vibration mode shapes of rigid pantograph-catenary system

下载: 全尺寸图片幻灯片

在刚柔过渡段处，地铁的运行速度v约为50 km/h，根据式（5）可以计算出此时弓网系统上接触线波磨波长λ=34.8 mm. 图6为实际运营线路上产生的接触线波磨，其波长约为36.0 mm，两者误差为3.3%，满足工程误差要求，由此可以证明此模型的准确性.

图 6 接触线波磨波长

Figure 6. Wavelength of contact wire corrugation

下载: 全尺寸图片幻灯片

$\lambda =\frac{v}{f} .$

(5)

基于本小节的工况参数，将逐个探究某一具体参数的变化对弓网系统摩擦自激振动的影响. 各个参数的变化范围如表4所示.

表 4 弓网系统计算参数变化范围

Table 4. Variation range of calculation parameters of pantograph-catenary system

参数	数值
摩擦系数	0.11～0.45
法向接触力/N	40～260
碳滑板接触位置	中间位置，位置 1～4
接触网类型	刚性接触网、刚柔过渡处、柔性接触网
弓头弹簧刚度/ （N•m⁻¹）	1100～5600

下载: 导出CSV

| 显示表格

2.2 摩擦系数对弓网系统摩擦自激振动的影响

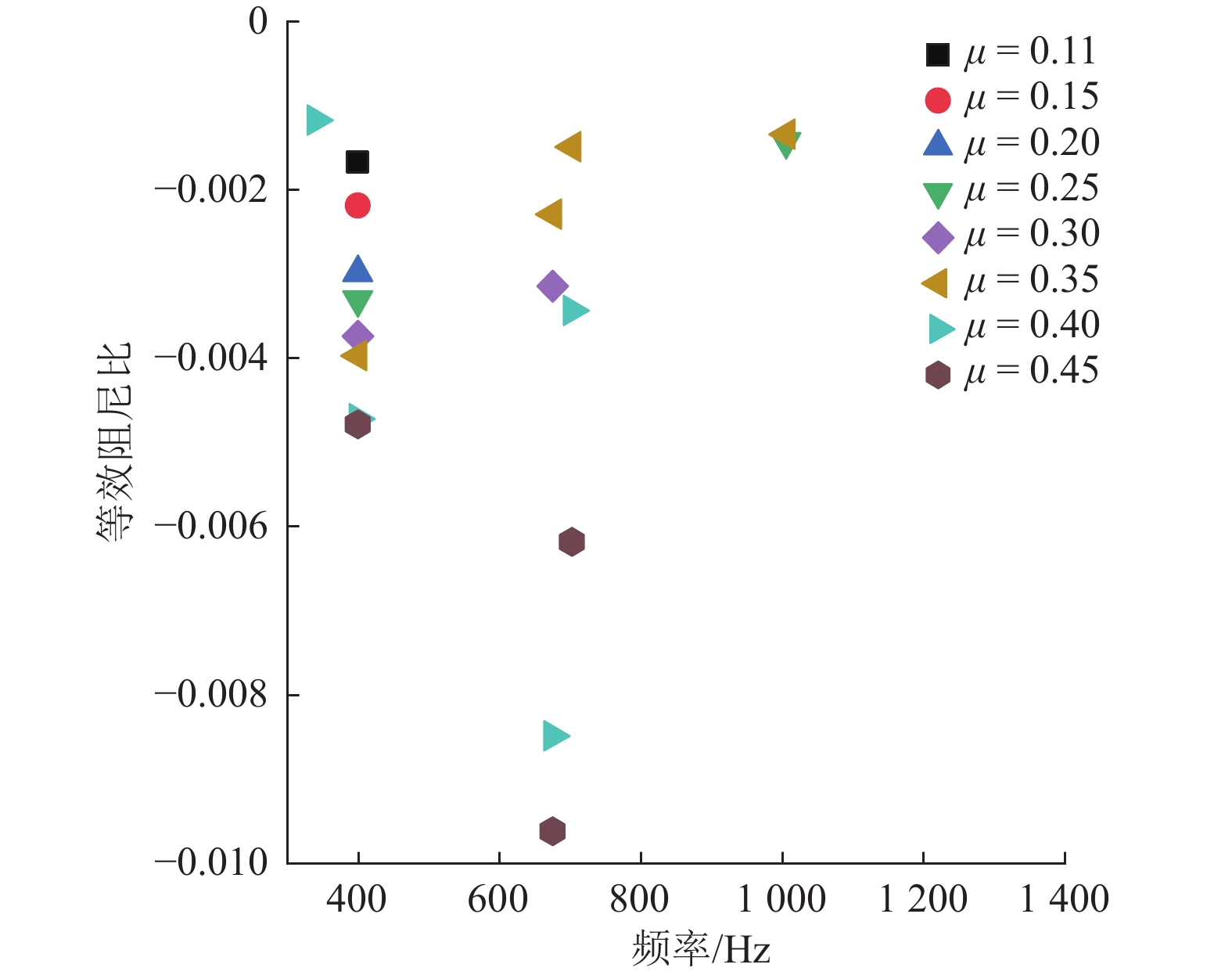
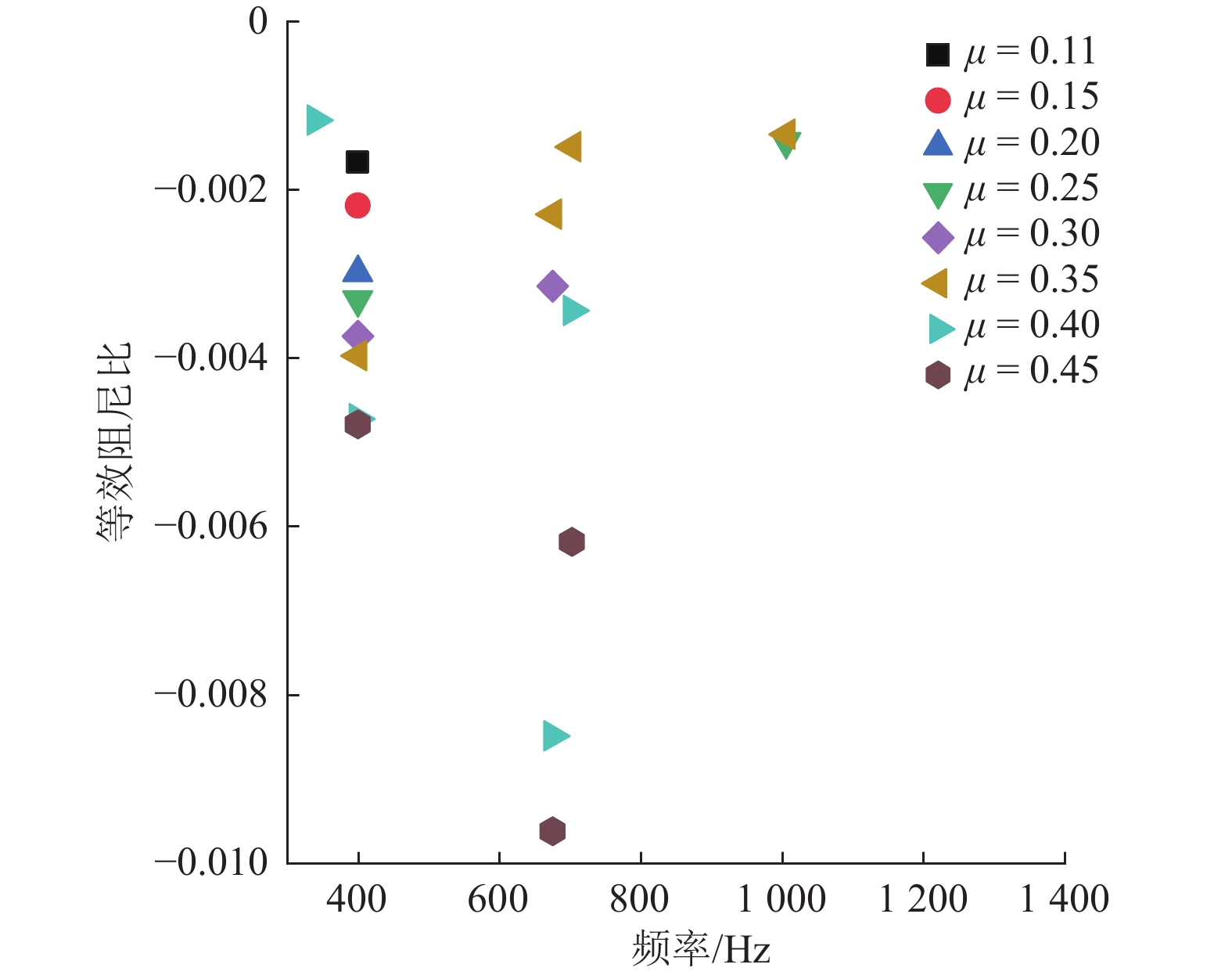
摩擦耦合是产生摩擦自激振动的一个重要因素，当摩擦系数越大，摩擦耦合就会越强，所以摩擦系数对弓网系统摩擦自激振动的产生有很大影响. 在地铁运行时，碳滑板与接触线的摩擦系数为0.25～0.36^[24]. 图7为不同摩擦系数下，不稳定振动频率和等效阻尼比的变化关系. 当μ < 0.11时，弓网系统没有出现摩擦自激振动；当摩擦系数从0.11增大到0.45时，频率399.61 Hz对应的等效阻尼比越来越小，弓网系统越容易发生摩擦自激振动；随着μ不断增大，系统出现不稳定振动频率的个数也在增加.

图 7 等效阻尼比和频率的变化关系

Figure 7. Variation of equivalent damping ratio with frequency under different friction coefficients

下载: 全尺寸图片幻灯片

2.3 法向接触力对弓网系统摩擦自激振动的影响

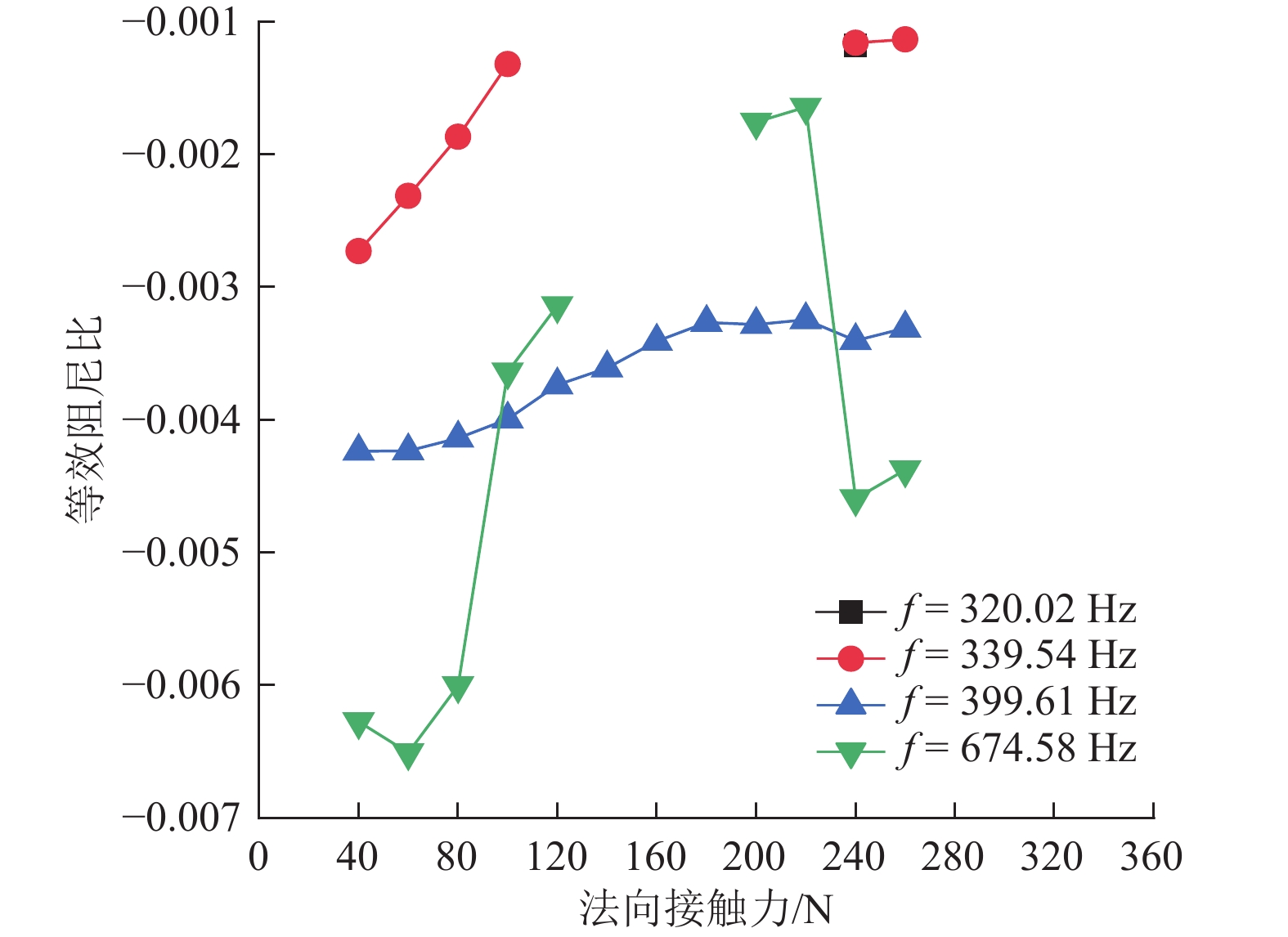
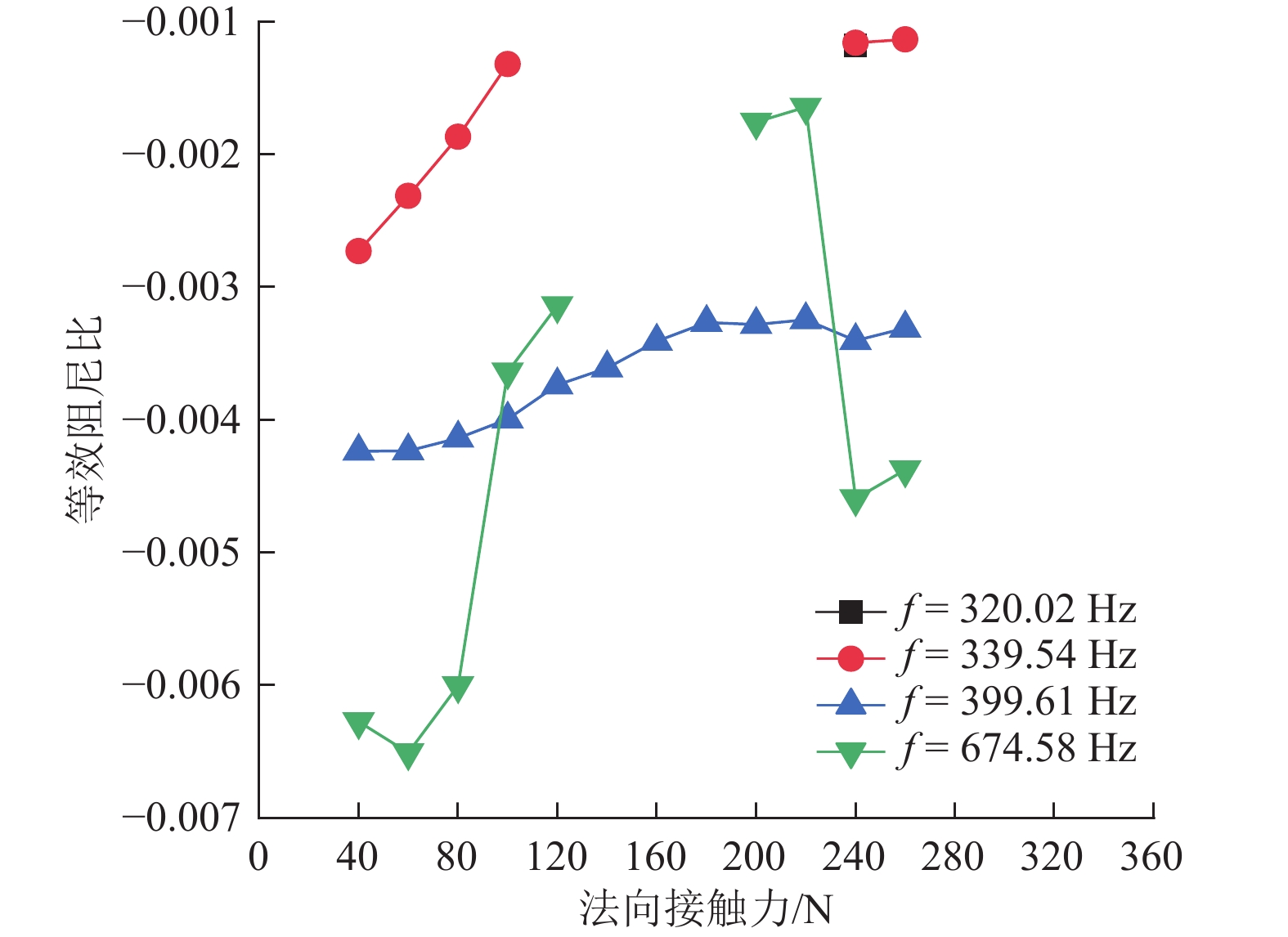
图8为系统不稳定振动频率对应的等效阻尼比随法向接触力的变化情况，从图8可以看出：当F在140～180 N内变化时，弓网系统只有一个频率为399.61 Hz的不稳定振动，且随着法向接触力的增加，该频率对应的等效阻尼比也在增大，系统摩擦自激振动得到减缓；当F为120、200、220 N时，只有2个不稳定振动频率，分别为399.61 Hz和674.58 Hz；当F为40～100 N以及260 N时，弓网系统出现了3个不稳定振动频率，分别为339.54、399.61、674.58 Hz；F = 240 N时，系统存在4个不稳定振动频率，此时系统的摩擦自激振动最为剧烈. 由此可以看出，法向接触力太大或者太小都不利于减缓弓网系统的摩擦自激振动，而在140～180 N内适当增加法向接触力，可以有效缓解弓网系统的摩擦自激振动.

图 8 系统不稳定振动频率对应的等效阻尼比随法向接触力的变化

Figure 8. Variation of equivalent damping ratio of unstable vibration frequency of system with normal contact force

下载: 全尺寸图片幻灯片

2.4 不同接触位置对弓网系统摩擦自激振动的影响

2.4.1 碳滑板不同接触位置对弓网系统摩擦自激振动的影响

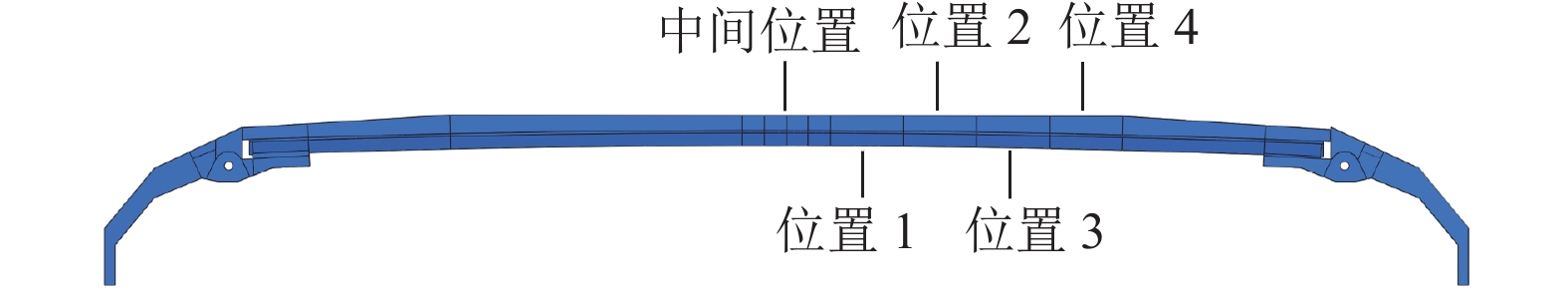
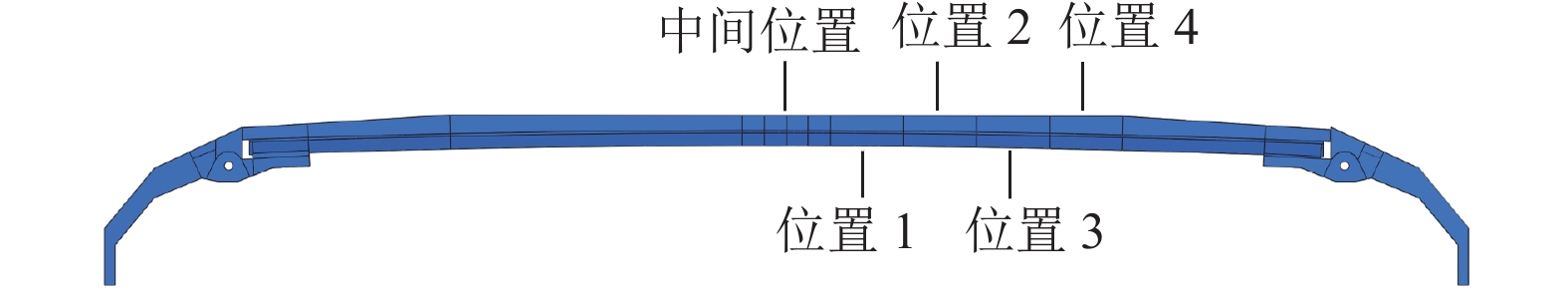
地铁正常运行时，受电弓上的碳滑板会随着列车的移动而与接触线在横向方向上发生相对滑动，导致碳滑板并不会只磨损中间位置，其实际磨损状况见图9. 从图中可以看出，碳滑板在整个接触面几乎都会产生磨损. 根据实际线路磨损位置，在有限元模型中将碳滑板与接触线的接触位置沿中间位置依次等距偏移了4次，见图10.

图 9 实际线路中碳滑板磨损位置

Figure 9. Wear position of a carbon strip in actual lines

下载: 全尺寸图片幻灯片

图 10 有限元模型中碳滑板接触位置

Figure 10. Contact position of carbon strip in finite element model

下载: 全尺寸图片幻灯片

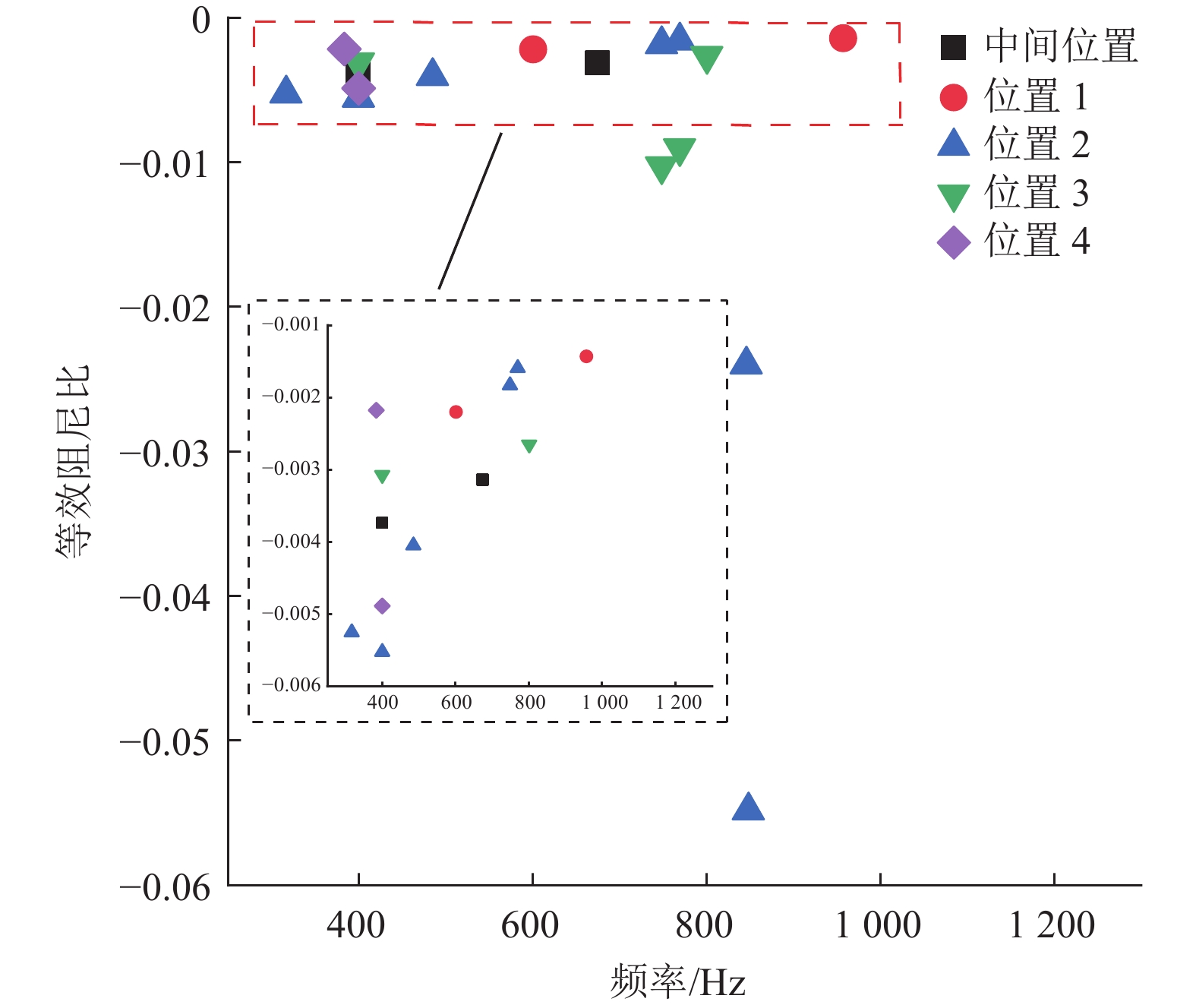
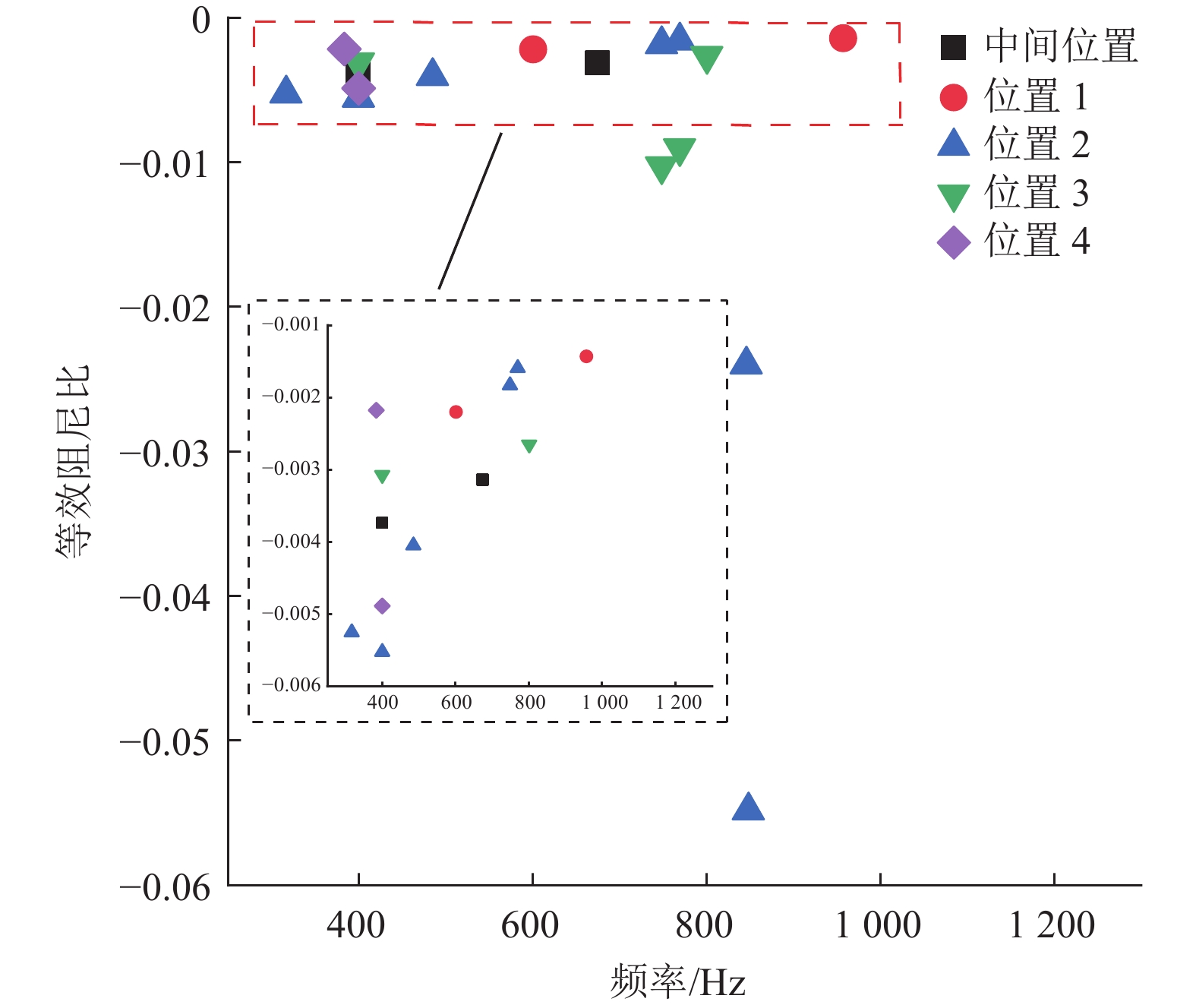
在有限元模型中对不同接触位置进行复特征值分析，位置不同时，等效阻尼比与频率的变化关系如图11所示. 从图11中可以看出：当碳滑板接触位置为中间位置、位置1和位置4时，弓网系统只存在2个不稳定振动频率；而当接触位置为位置2和3时，弓网系统分别出现了7个和4个不稳定振动频率，且在位置2的区域时，出现了阻尼比急剧变小的不稳定振动频率，导致系统不稳定振动发生的趋势增大. 由此可以看出：当碳滑板接触位置不同时，对弓网系统的摩擦自激振动有较大的影响，改变碳滑板的接触位置并不能消除弓网系统的不稳定振动，并且在位置2接触时会使系统的不稳定振动更加剧烈，接触网的布置应当考虑减少甚至避免出现位置2与接触线长期耦合摩擦的情况.

图 11 碳滑板接触位置变化，等效阻尼比与频率的变化关系

Figure 11. Variation of contact position of carbon strip and variation of equivalent damping ratio with frequency

下载: 全尺寸图片幻灯片

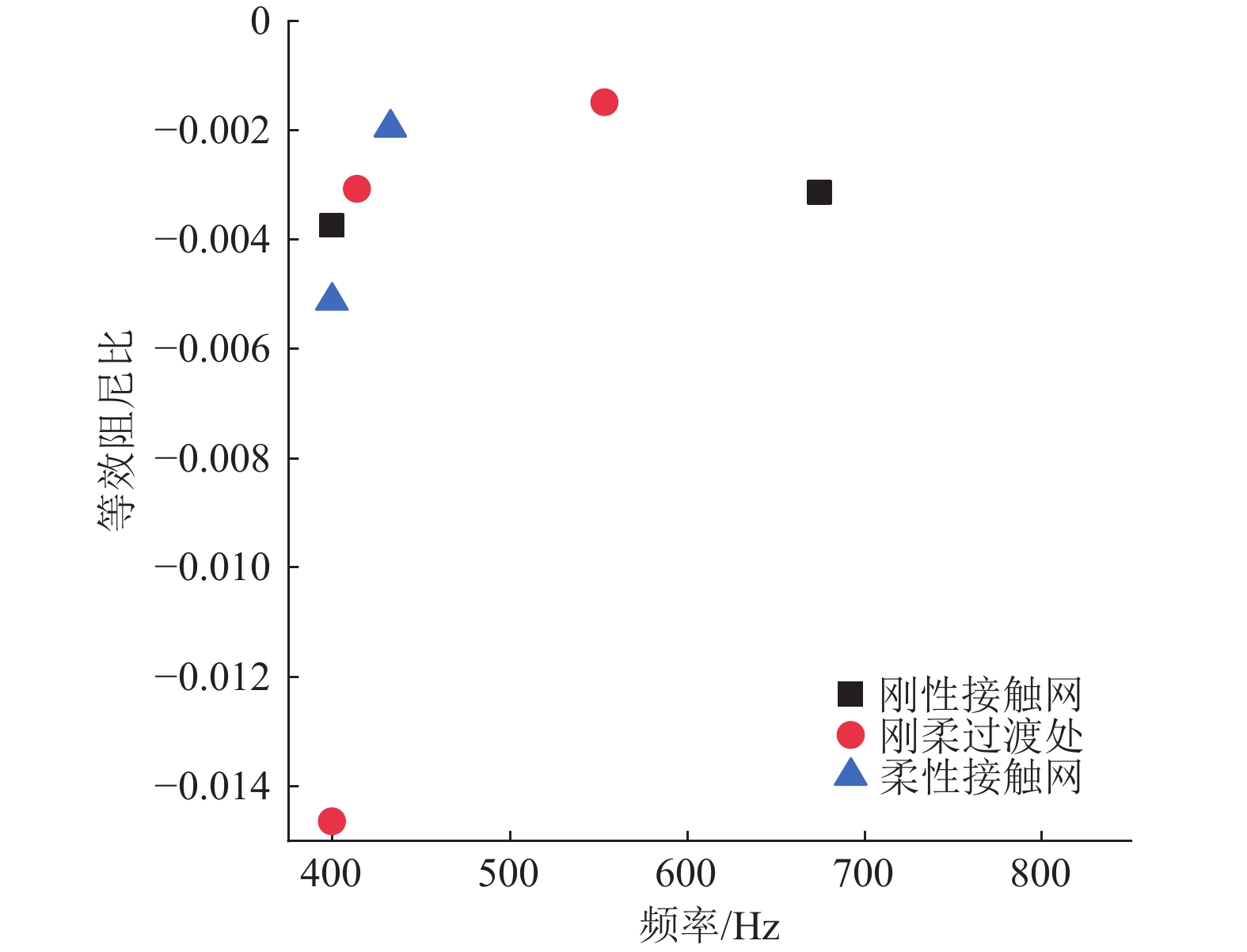
2.4.2 不同接触网对弓网系统摩擦自激振动的影响

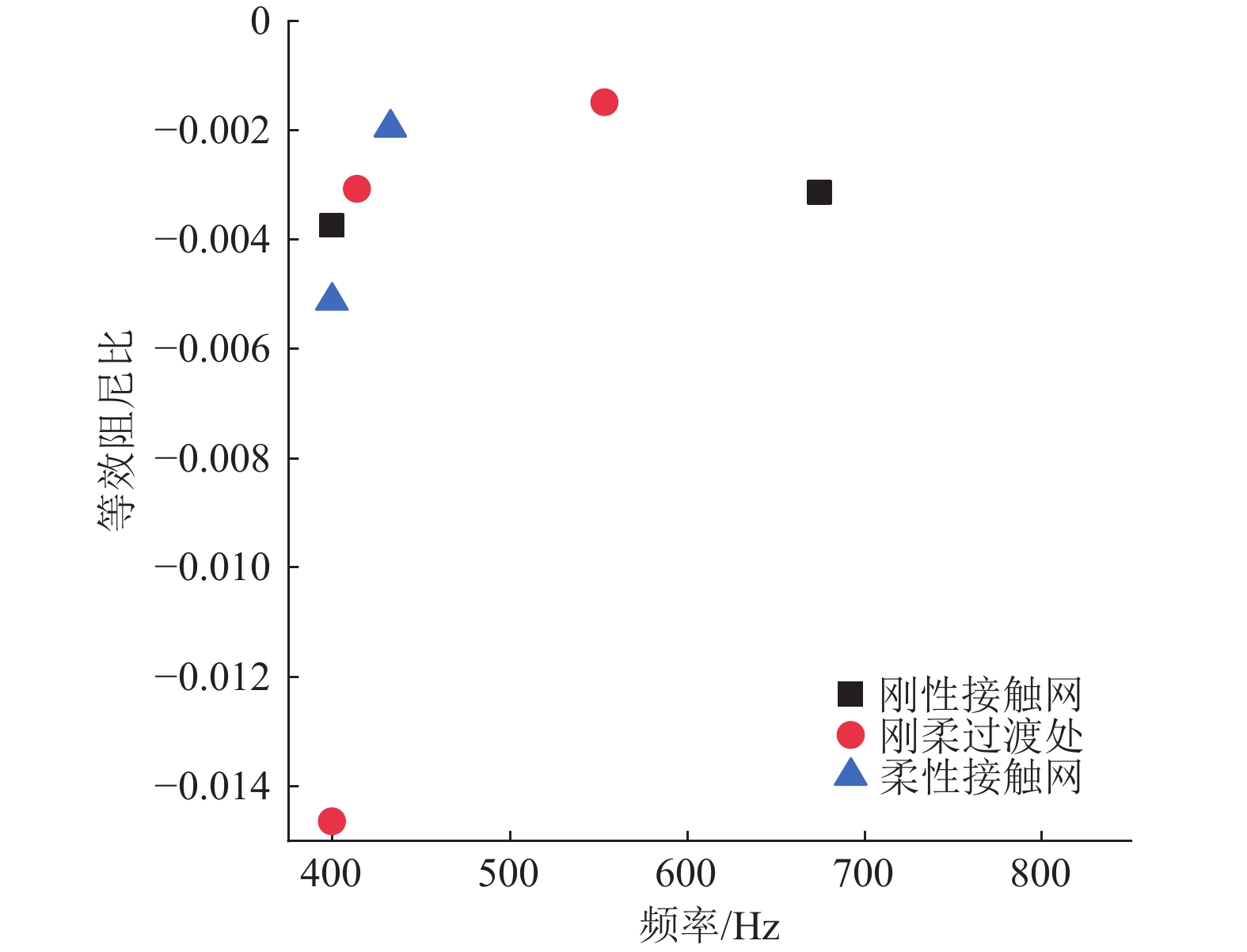
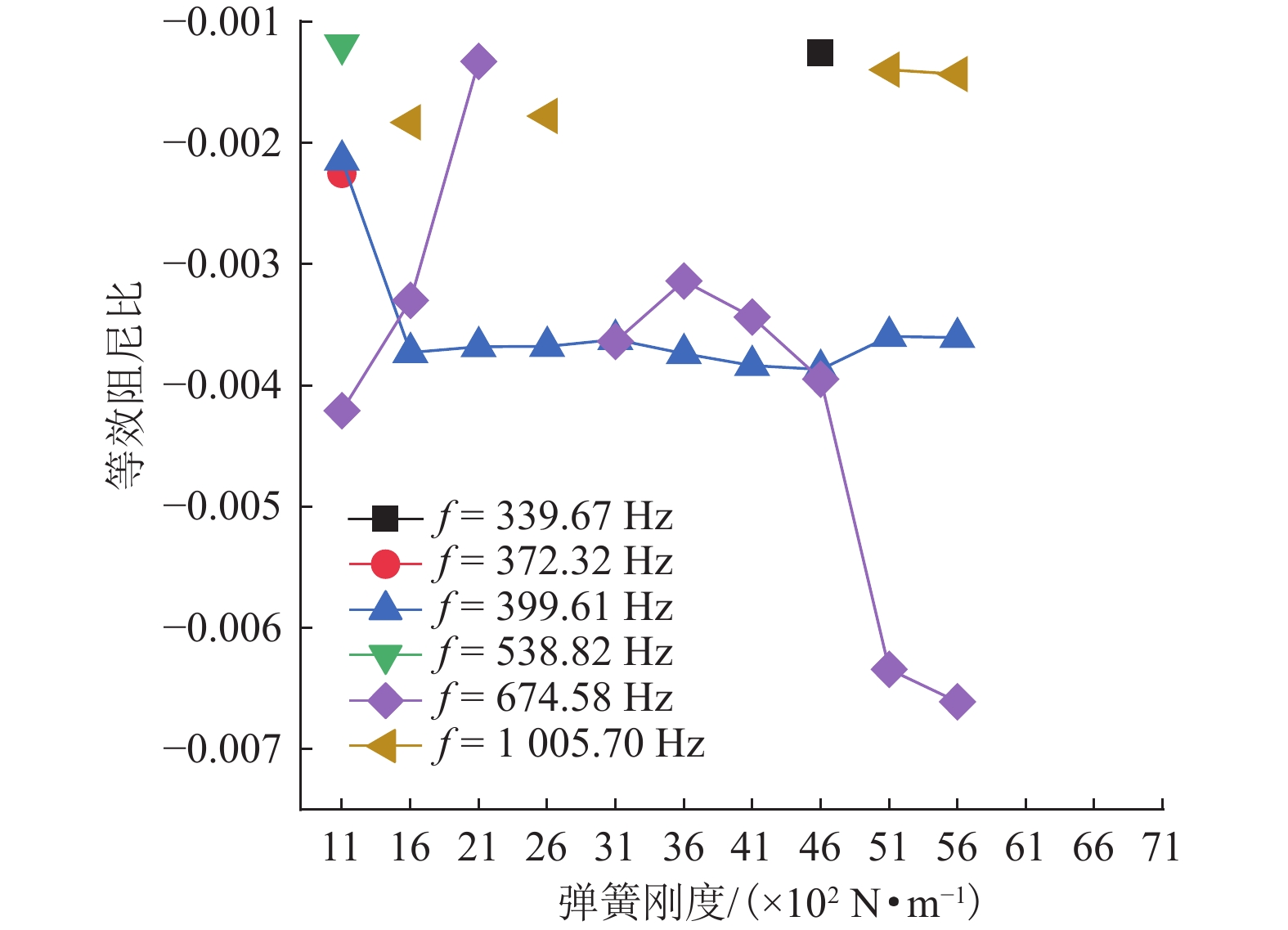
当受电弓滑过同一类型的接触网时，弓网系统的摩擦自激振动不会发生变化，只会改变摩擦自激振动发生的可能性^[15]. 但很少有学者研究受电弓滑过不同接触网时对弓网系统摩擦自激振动的影响. 因此，本小节将受电弓分别放置于刚性接触网、刚柔过渡结构处以及柔性接触网处，对模型进行复特征值分析，得到的结果如图12所示. 由图12可以看出：当受电弓滑过不同类型的接触网时，其摩擦自激振动的主频都是399.61 Hz，且刚性接触网最为稳定；而在刚柔过渡结构处弓网系统存在3个不稳定振动频率，且主频399.61 Hz对应的等效阻尼比ζ=−1.464 × 10⁻²是三者之间的最小值. 因此，弓网系统在刚柔过渡结构处最容易发生摩擦自激振动.

图 12 不同接触网位置下，等效阻尼比与频率的变化关系

Figure 12. Variation of equivalent damping ratio with frequency under different catenary positions

下载: 全尺寸图片幻灯片

2.5 弓头弹簧刚度对弓网系统摩擦自激振动的影响

合适的弓头弹簧刚度有利于弓网系统保持稳定的受流质量. 本节利用复特征值分析方法计算了不同弓头弹簧刚度对弓网系统摩擦自激振动的影响. 从图13中可以看出：弓头弹簧刚度从1100 N/m增大到5600 N/m时，弓网系统摩擦自激振动的主频399.61 Hz一直存在；当弹簧刚度小于2100 N/m和大于4100 N/m时，弓网系统不稳定振动频率的数量为3～4个，且都存在674.58 Hz的不稳定振动频率；当弹簧刚度过大或者过小时，频率674.58 Hz对应的等效阻尼比也在减小. 可见，弹簧刚度过大或者过小更容易导致弓网系统发生摩擦自激振动；当弹簧刚度在2100～4100 N/m内时，弓网系统较为稳定，都只存在2个不稳定振动频率.

图 13 不同频率下，等效阻尼比ζ与弓头弹簧刚度的变化关系

Figure 13. Variation of equivalent damping ratio with stiffness of pantograph bow spring under different frequencies

下载: 全尺寸图片幻灯片

3. 结　论

本文建立了地铁刚柔过渡段弓网系统有限元模型，主要对刚性接触网的摩擦自激振动进行了参数敏感性分析，并且研究了受电弓处于不同接触网位置时弓网系统的摩擦自激振动. 针对此弓网系统，得出了以下主要结论：

1）当μ ≥ 0.11时，弓网系统将产生摩擦自激振动现象，其主频为399.61 Hz. 当μ < 0.11时，可以抑制甚至消除弓网系统摩擦自激振动引起的接触损耗.

2）法向接触力在140～180 N内变化可以有效缓解弓网系统的摩擦自激振动，而法向接触力太大或者太小都会导致弓网系统的摩擦自激振动更加剧烈.

3）碳滑板的不同接触位置并不能完全消除弓网系统的摩擦自激振动，且位置2和位置3的摩擦自激振动现象更为明显. 当受电弓位于刚性接触网时，弓网系统的稳定性优于其他接触网，而当受电弓位于刚柔过渡结构处时，弓网系统最不稳定，最容易发生摩擦自激振动.

4）弓头弹簧刚度在2100～4100 N/m内时，弓网系统较为稳定.

图 1 接触网有限元模型

Figure 1. Finite element model of catenary

下载: 全尺寸图片幻灯片

图 2 柔性接触网模型

Figure 2. Flexible catenary model

下载: 全尺寸图片幻灯片

图 3 受电弓有限元模型

Figure 3. Finite element model of pantograph

下载: 全尺寸图片幻灯片

图 4 弓网系统有限元模型

Figure 4. Finite element model of pantograph-catenary system

下载: 全尺寸图片幻灯片

图 5 刚性弓网系统不稳定振动模态

Figure 5. Unstable vibration mode shapes of rigid pantograph-catenary system

下载: 全尺寸图片幻灯片

图 6 接触线波磨波长

Figure 6. Wavelength of contact wire corrugation

下载: 全尺寸图片幻灯片

图 7 等效阻尼比和频率的变化关系

Figure 7. Variation of equivalent damping ratio with frequency under different friction coefficients

下载: 全尺寸图片幻灯片

图 8 系统不稳定振动频率对应的等效阻尼比随法向接触力的变化

Figure 8. Variation of equivalent damping ratio of unstable vibration frequency of system with normal contact force

下载: 全尺寸图片幻灯片

图 9 实际线路中碳滑板磨损位置

Figure 9. Wear position of a carbon strip in actual lines

下载: 全尺寸图片幻灯片

图 10 有限元模型中碳滑板接触位置

Figure 10. Contact position of carbon strip in finite element model

下载: 全尺寸图片幻灯片

图 11 碳滑板接触位置变化，等效阻尼比与频率的变化关系

Figure 11. Variation of contact position of carbon strip and variation of equivalent damping ratio with frequency

下载: 全尺寸图片幻灯片

图 12 不同接触网位置下，等效阻尼比与频率的变化关系

Figure 12. Variation of equivalent damping ratio with frequency under different catenary positions

下载: 全尺寸图片幻灯片

图 13 不同频率下，等效阻尼比ζ与弓头弹簧刚度的变化关系

Figure 13. Variation of equivalent damping ratio with stiffness of pantograph bow spring under different frequencies

下载: 全尺寸图片幻灯片

表 1 刚性接触网模型参数

Table 1. Rigid catenary model parameters

部件	材料	密度/ （× 10³ kg•m⁻³）	弹性模量/ GPa	泊松比
汇流排	6101B	2.71	69	0.33
接触线	CTHA120	8.92	130	0.30
定位线夹	铝合金	2.70	70	0.34
绝缘子	陶瓷	3.80	340	0.22

下载: 导出CSV

表 2 柔性接触网模型参数

Table 2. Flexible catenary model parameters

部件	材料	密度/ （× 10³ kg•m⁻³）	弹性模量/ GPa	泊松比
承力索	JT150	9.20	105	0.30
接触线	CTHA120	8.92	130	0.30

下载: 导出CSV

表 3 受电弓各部件材料

Table 3. Material properties of pantograph components

部件	材料	密度/ （ × 10³ kg•m^–3）	弹性模量/ GPa	泊松比
平衡杆	碳纤维	1.9	231	0.23
碳滑板	铜、碳	2.4	12.6	0.43
下臂杆	铝合金	2.8	72	0.33
弓头支架	钛合金	4.5	117	0.34
其他部件	Q235	7.8	210	0.30

下载: 导出CSV

表 4 弓网系统计算参数变化范围

Table 4. Variation range of calculation parameters of pantograph-catenary system

参数	数值
摩擦系数	0.11～0.45
法向接触力/N	40～260
碳滑板接触位置	中间位置，位置 1～4
接触网类型	刚性接触网、刚柔过渡处、柔性接触网
弓头弹簧刚度/ （N•m⁻¹）	1100～5600

下载: 导出CSV

参考文献(24)

[1]	关金发,田志军,吴积钦. 基于弓网动力仿真的160 km/h刚柔过渡系统方案研究[J]. 铁道学报,2018,40(9): 48-56. GUAN Jinfa, TIAN Zhijun, WU Jiqin. Research of 160 km/h transition structure proposal between overhead conductor railand contact line based on dynamic simulation[J]. Journal of the China Railway Society, 2018, 40(9): 48-56.
[2]	王建红. 重庆轨道六号线支线二期工程接触网刚柔过渡[J]. 现代制造技术与装备,2022,58(9): 127-129. WANG Jianhong. Rigid flexible transition of OCS of Chongqing rail line 6 branch line phase Ⅱ project[J]. Modern Manufacturing Technology and Equipment, 2022, 58(9): 127-129.
[3]	杨清太. 既有线铁路接触网刚柔过渡问题探析[J]. 工程建设与设计,2021(13): 74-76. YANG Qingtai. A study on rigid and flexible catenary transition for existing rail-line[J]. Construction & Design for Engineering, 2021(13): 74-76.
[4]	梅桂明. 刚性接触网-受电弓载流磨损性能的试验研究[J]. 西南交通大学学报,2021,56(6): 1305-1310. MEI Guiming. Experimental study on wear performance of rigid catenary-pantograph system with direct current[J]. Journal of Southwest Jiaotong University, 2021, 56(6): 1305-1310.
[5]	周宁,支兴帅,张静,等. 电气化铁路弓网系统摩擦磨损性能研究进展[J]. 西南交通大学学报,2024,59(5):990-1005. ZHOU Ning, ZHI Xingshuai, ZHANG Jing, et al. Friction and wear performance of pantograph-catenary system in electrified railways: State of the art[J]. Journal of Southwest Jiaotong University, 2024, 59(5):990-1005.
[6]	范杰,刘达毅,董丙杰,等. 地铁刚性弓网系统接触线磨损特性试验研究[J]. 润滑与密封,2022,47(6): 45-51. FAN Jie, LIU Dayi, DONG Bingjie, et al. Experimental study on the wear characteristics of contact wire of metro rigid pantograph-catenary systems[J]. Lubrication Engineering, 2022, 47(6): 45-51.
[7]	YANG H J, LI C, LIU Y H, et al. Study on the delamination wear and its influence on the conductivity of the carbon contact strip in pantograph-catenary system under high-speed current-carrying condition[J]. Wear, 2021, 477:203823.1-203823.14.
[8]	MANDAI T, HARADA S, SHIMIZU M, et al. Improvement of rigid conductor lines[J]. Quarterly Report of RTRI, 2003, 44(2): 78-81. doi: 10.2219/rtriqr.44.78
[9]	WU T X, BRENNAN M J. Dynamic stiffness of a railway overhead wire system and its effect on pantograph-catenary system dynamics[J]. Journal of Sound and Vibration, 1999, 219(3): 483-502. doi: 10.1006/jsvi.1998.1869
[10]	CHEN L, LIU Z G, ZHANG J, et al. Influence of key parameters on high speed overhead conductor rail and pantograph system[C]//The IAVSD International Symposium on Dynamics of Vehicles on Roads and Tracks. Cham: Springer, 2020: 201-211.
[11]	SHIMIZU M, HARADA S, OYA A, et al. Improving performance of type T overhead rigid conductor lines[J]. Quarterly Report of RTRI, 2006, 47(1): 52-58. doi: 10.2219/rtriqr.47.52
[12]	钱世勇,陈珍宝,陈明国,等. 地铁弓网动态试验研究及分析[J]. 科协论坛(下半月),2012(5): 26-27. QIAN Shiyong, CHEN Zhenbao, CHEN Mingguo, et al. Research and analysis on dynamic test of metro pantograph and catenary[J]. Science & Technology Association Forum, 2012(5): 26-27.
[13]	谭冬华. 架空刚性接触悬挂弓网磨耗异常的解决办法[J]. 都市快轨交通,2007,20(2): 88-91. TAN Donghua. Causes and solutions for the abnormal pantograph-catenary attrition of rigid catenary[J]. Urban Rapid Rail Transit, 2007, 20(2): 88-91.
[14]	裴志禹. AC25 kV刚性接触网过渡段结构优化研究[D]. 成都:西南交通大学,2018.
[15]	QIAN W J, CHEN G X, ZHANG W H, et al. Friction-induced, self-excited vibration of a pantograph-catenary system[J]. Journal of Vibration and Acoustics, 2013, 135(5): 051021.1- 051021. 8
[16]	WU B W, SHANG Z Y, PAN J B, et al. Analysis on the formation cause for the high-order wheel polygonization of the high-speed trains based on the finite element method[J]. Vehicle System Dynamics, 2023, 61(1): 1-18. doi: 10.1080/00423114.2022.2035777
[17]	CUI X L, CHENG Z, YANG Z C, et al. Study on the phenomenon of rail corrugation on high-speed rail based on the friction-induced vibration and feedback vibration[J]. Vehicle System Dynamics, 2022, 60(2): 413-432. doi: 10.1080/00423114.2020.1817507
[18]	康熙,陈光雄,吕金洲,等. 缩尺轮轨模型中钢轨波磨的相似性[J]. 西南交通大学学报,2020,55(6): 1320-1327. KANG Xi, CHEN Guangxiong, LÜ Jinzhou, et al. Similarity of small-scale wheelset-track model for investigation of rail corrugation[J]. Journal of Southwest Jiaotong University, 2020, 55(6): 1320-1327.
[19]	陈光雄. 钢轨波磨预测模型验证工况的研究[J]. 西南交通大学学报,2022,57(5): 1017-1023,1054. CHEN Guangxiong. Study on validation conditions of rail corrugation prediction models[J]. Journal of Southwest Jiaotong University, 2022, 57(5): 1017-1023,1054.
[20]	田珂. 地铁受电弓—刚性接触网建模及仿真研究[D]. 成都:西南交通大学,2018.
[21]	朱志增. 基于有限元分析的刚性悬挂弓网接触压力仿真研究[D]. 成都:西南交通大学,2014.
[22]	杨艺,周宁,李瑞平,等. 基于有限元法的弓网过渡段处动态性能仿真分析[J]. 振动与冲击,2016,35(18): 71-75,116. YANG Yi, ZHOU Ning, LI Ruiping, et al. Dynamic performance analysis of different sections of overhead catenary based on finite element model[J]. Journal of Vibration and Shock, 2016, 35(18): 71-75,116.
[23]	YUAN Y. An eigenvalue analysis approach to break squeal problem[C]// Proceedings of the 29th ISATA conference on automotive braking systems. Florence: ISATA, 1996: 3-6.
[24]	黄之元. 两种地铁刚性接触网摩擦副异常磨耗的试验研究[D]. 成都:西南交通大学,2020.