Anti-Disturbance Performance of Maglev Rotor Using Model Assisted Extended State Observer
-
摘要:
随着正弦干扰频率的提高,扩张状态观测器(extended state observer,ESO)的性能会下降,为提高磁悬浮转子系统中ESO的干扰抑制能力,首先,建立单自由度磁悬浮轴承转子系统数学模型;其次,设计ESO并分析其干扰抑制效果下降的原因;在此基础上,提出一种模型辅助扩张状态观测器(model assisted extended state observer, MESO)以改进带宽配置方式,提高干扰抑制效果;然后,在频域内分析基于MESO的自抗扰控制器的稳定性;最后,通过仿真与试验验证了所提出观测器的有效性. 研究结果表明:带宽的增加会放大系统噪声的影响,使系统的控制电压增加;随着干扰频率的提高,MESO对高频正弦干扰的抑制效果会下降,但仍可以降低转子的模态振幅;对50 Hz旋转频率下的转子分别施加频率为10 Hz、振幅为2 mm的基础简谐干扰与1
g 的基础冲击干扰, 相比ESO,MESO控制下的转子位移分别降低了16.3%与22.6%,控制电压降低了约14%.Abstract:With the increase in sinusoidal disturbance frequency, the performance of extended state observers (ESOs) will decrease. In order to improve the disturbance suppression ability of the ESO in the maglev rotor system, firstly, the mathematical model of a one-degree-of-freedom (1-DOF) maglev bearing rotor system was built. Secondly, ESO was designed, and the reasons for its reduced disturbance suppression effects were analyzed. On this basis, a model assisted ESO (MESO) was proposed to improve the bandwidth configuration and enhance the disturbance suppression effects. Then, the stability of the active disturbance rejection controller based on MESO was analyzed in the frequency domain. The effectiveness of the proposed observer was finally verified through simulation and experiments. The research results indicate that an increase in bandwidth amplifies the impact of system noises and increases the control voltage of the system. As the disturbance frequency increases, the suppression effect of MESO on high-frequency sinusoidal disturbance will decrease, but it can still reduce the modal amplitude of the rotor. After applying fundamental harmonic disturbance of 10 Hz−2 mm and fundamental impulse disturbance of 1
g to the rotor at a rotating frequency of 50 Hz respectively, the rotor displacement under MESO control is reduced by 16.3% and 22.6%, respectively compared with that under ESO control, and the control voltage is reduced by about 14%. -
目前,主动磁悬浮轴承(active magnetic bearing, AMB)受到了广泛的关注,具有巨大的经济效益和广阔的应用前景,逐渐成为研究热点之一. 与传统机械轴承相比,AMB具有无机械接触、长使用寿命、高旋转速度、可主动控制等优点[1],在人工心脏泵[2]、高速电机[3]等领域被广泛使用.
由于具有负刚度特性,AMB转子系统本质上是不稳定的,需要引入闭环控制回路保证其稳定性. 目前已有多种控制方法被应用于AMB转子系统中,如自适应控制[4]、H∞控制[5]等. 因为实现成本较高等原因,上述控制算法没有在工业中得到广泛应用. PID 控制器(proportional-integral-derivative controller)凭借其结构简单,参数调整方便等优势,是目前工业中应用最多的控制算法. 然而,AMB转子系统在运行中会受到多种干扰的影响,有转子质量不平衡引起的转速同频干扰,基础振动引起的低频干扰[6],传感器跳动引起的多频干扰[7],还包括系统参数摄动和未建模动态等内部干扰[8]. 干扰的存在会影响系统的稳定性,因此,AMB控制器必须具有一定的抗干扰能力以保证其稳定运行.
为了提高传统PID控制器的抗干扰能力,Han[9]在90年代提出了自抗扰控制(active disturbance rejection control, ADRC),其核心为PID控制器与ESO(extended state observer). ADRC不仅具有PID控制器结构简单、参数调整方便的优点,还具有很好的抗干扰能力. 目前,ADRC在磁悬浮领域已有很多的应用[10-11]. 然而,ADRC控制器的性能主要取决于ESO对于系统状态与外部干扰估计的准确性. Guo等[12]证明了ESO对于常数扰动能够实现渐进收敛. Zheng等[13]认为当系统不确定性的变化率有界时,ESO的估计误差也保持有界. 理论上,ESO的带宽越大,干扰估计的效果越好,但同时采样噪声对于系统的影响也越大,因此,带宽的选择需要合理平衡噪声影响和干扰抑制效果[14]. Godbole等[15]分析了ESO对正弦干扰的估计效果,结果表明,当带宽明显大于扰动频率时ESO才能对干扰进行有效观测,但是带宽并不能无上限地增加. 因此,干扰频率越大,相同带宽下ESO的观测效果就越差.
为了提高ESO的干扰估计效果,本文在ESO的基础上提出MESO,改进了ESO的带宽配置方式. 在ESO的干扰估计回路中引入模型信息进行辅助,使MESO (model assisted extended state observer)在同样的带宽下具有更大的观测器增益,从而提高了ESO的干扰估计效果,为实际工程中ESO的应用与调试提供指导.
1. 磁悬浮轴承转子系统数学建模
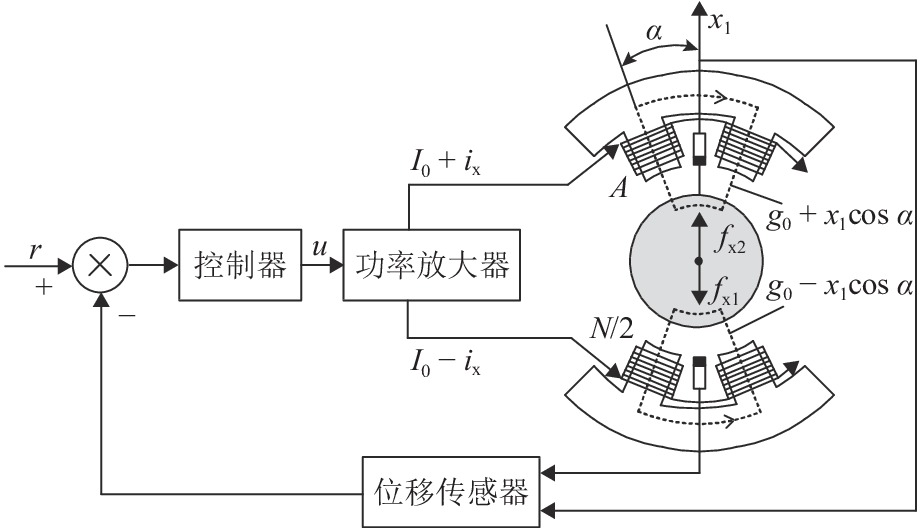
AMB在5个自由度上的工作原理相同,所以本文对单自由度AMB转子系统进行分析. 图1为单自由度AMB转子系统结构,其中,磁悬浮轴承采用8极C型的结构. 图中:α、A、N分别为磁极夹角、磁极面积与一对磁极上的线圈匝数;r为转子参考位移;I0、ix分别为偏置电流、控制电流;fx1、fx2分别为两个电磁铁产生的电磁力;g0为转子的平衡间隙;u为控制电压. 当转子的位置发生偏移时, g0会发生变化,位移传感器实时监测转子位移,控制器根据转子位移与r的差值计算合适的u,再经过功率放大器变成控制电流ix,使一端电磁铁上的电流为I0与ix之和,另一端为I0与ix之差,从而分别改变f1、f2的大小,通过差动控制使转子稳定悬浮在给定位置.
本文研究的AMB转子系统工作转速低于0.7倍一阶弯曲临界转速,可视为刚性转子进行建模. 忽略不同自由度之间的耦合作用,根据牛顿第二定律,可以写出单自由度磁悬浮轴承-转子系统的传递函数,如式(1).
X(s)I(s)=kikaksms2−kx, (1) 式中:X(s)与I(s)为位移x1与电流i的拉普拉斯变换表达式;kx为位移刚度系数, kx=cos2α((μ0N2AI02)/g30);ki为电流刚度系数, ki=cosα((μ0N2AI0)/g20);m为转子质量;ks为位移传感器增益;ka为功率放大器增益; μ0为真空磁导率.
假设系统受到的外部干扰为f,转子的位移为x1,则式(1)的状态空间方程为
{x2=˙x1,˙x2=kikaksmu+kxmx1+fm,y=x1. (2) 2. 模型辅助ESO的设计
ESO最初提出时为非线性结构[9],但是由于线性扩张状态观测器(linear extended state observer, LESO)易于设计和实现而被广泛采用[16],因此,本文基于LESO进行研究.
2.1 ESO的设计
一个二阶线性系统的模型如式(3)所示.
{˙x=Ax+Bu,y=Cx+Du, (3) 式中:x、y均为系统的状态变量,x为位移向量;u为电压状态变量向量;A、B、C、D为状态空间的系数矩阵.
构造龙伯格观测器对系统的状态进行估计,其结构为
{˙z=Az+Bu+L(y−ˆy),ˆy=Cz+Du, (4) 式中: z、 ˆy均为系统状态观测值;L为观测器系数矩阵.
式(4)可化简为
˙z=(A−LC)z+(B−LD)u+Ly. (5) 对于二阶的磁悬浮轴承转子系统,将控制电压以外的量扩张为新的状态变量x3,如式(6),将其称作广义扰动fg.
x3=kxmx1+fm. (6) 则式(2)为
{x2=˙x1,˙x2=kikaksmu+x3,h=˙x3,y=x1. (7) 根据式(5)可以写出龙伯格观测器下系统状态变量的观测值为
{˙z1=−L1e1+z2,˙z2=−L2e1+z3+kikaksmu,˙z3=−L3e1, (8) 式中:L1、L2、L3为观测器的增益;z1、z2、z3分别为位移、加速度、广义扰动观测值;e1=z1−x1,为观测误差.
将式(8)与式(7)作差可得ESO的误差状态方程为
˙e=Aee−Eh, (9) 式中: Ae=[−L110−L201−L300]; E=[001]; e=[e1e2e3],e2=z2-x2,
e3为干扰误差,e3=z3-x3. 根据有界输入有界输出定理,因为Eh是有界的,所以系统的稳定性由Ae决定,Ae的特征方程为
|Ae|=|λ+L1−10L2λ−1L30λ|=λ3+L1λ2+L2λ+L3. (10) 为了保证系统的稳定性,令|Ae|=(λ + ω)3,ω为ESO的带宽,从而使得Ae的极点都在负半平面内,可得
[L1L2L3]=[3ω3ω2ω3]. (11) 将式(9)进行拉普拉斯变换,可以得到从干扰估计误差e3到广义扰动fg的传递函数为
e3fg=−s3+L1s2+L2ss3+L1s2+L2s+L3. (12) 将式(11)代入式(12)中可得
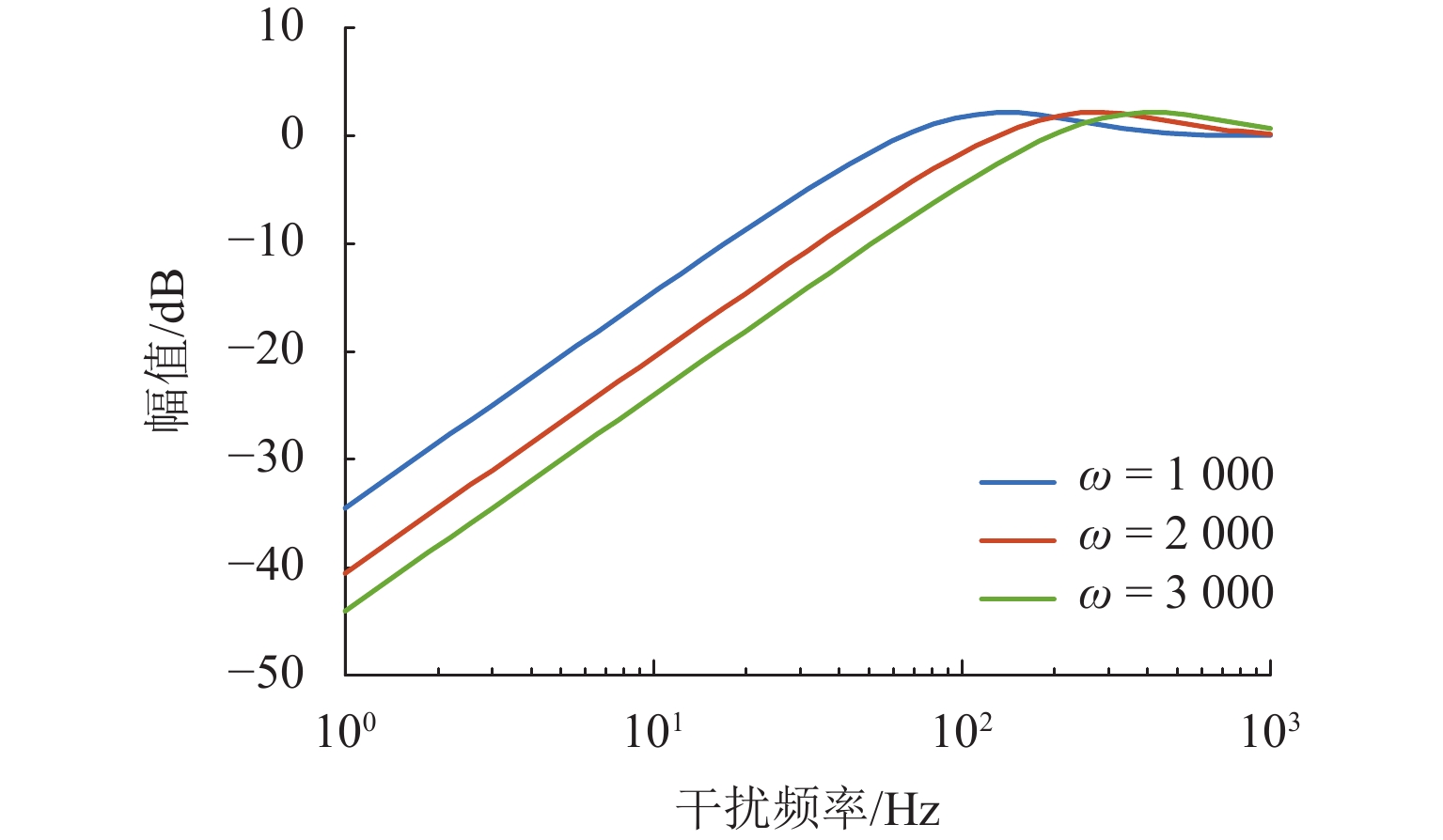
H1(s)=−s3+3ωs2+3ω2ss3+3ωs2+3ω2s+ω3. (13) 绘制H1(s)的伯德图如图2所示,其中,ω分别为1000、2000、3000. 从图中可以看出:带宽越大,观测器对干扰的抵消能力也越高;但是ω的提高也是有限制的,实际上带宽越大系统对噪声也越敏感;随着干扰频率的增大,ESO的干扰抑制效果逐渐降低,当干扰频率达到110 Hz时,ESO对外部干扰几乎没有抑制效果.
2.2 MESO的设计
为了提高ESO的干扰抵消能力,本文在ESO的基础上引入系统的模型信息,提出了MESO. 式(6)中,kxx1/m可以通过测量获得,将其作为已知信息,式(7)可以改写为
{˙x1=x2,˙x2=kikaksmu+x3,˙x3=kxm˙x1+h,y=x1. (14) 因此,MESO的观测方程为
{˙z1=z2−L1e1,˙z2=z3−L2e1+kikaksmu,˙z3=kxmz2−L3e1. (15) 将式(15)与式(14)作差可得MESO的误差状态方程为
˙e=Ae1e−Eh, (16) 式中:
Ae1=[−L110−L201−L3kx/m0]. 同样的,为了保证系统的稳定性,令|Ae1|=(λ + ω)3,使Ae1的极点全部配置于λ=−ω,可得
[L1 L2 L3]=[3ω3ω2+kxmω3+3ωkxm]. (17) 可以看出,与式(11)相比,因为加入了已知的模型信息(kx/m)x1项,所以MESO相比于ESO可以在相同的带宽ω下具有更大的增益,因此,MESO具有更好的观测效果. 对于MESO,从干扰估计误差e3到广义扰动fg的传递函数为
e3fg=−s3+L1s2+L2ss3+L1s2+L2s+L3. (18) 将式(17)代入(18)可得
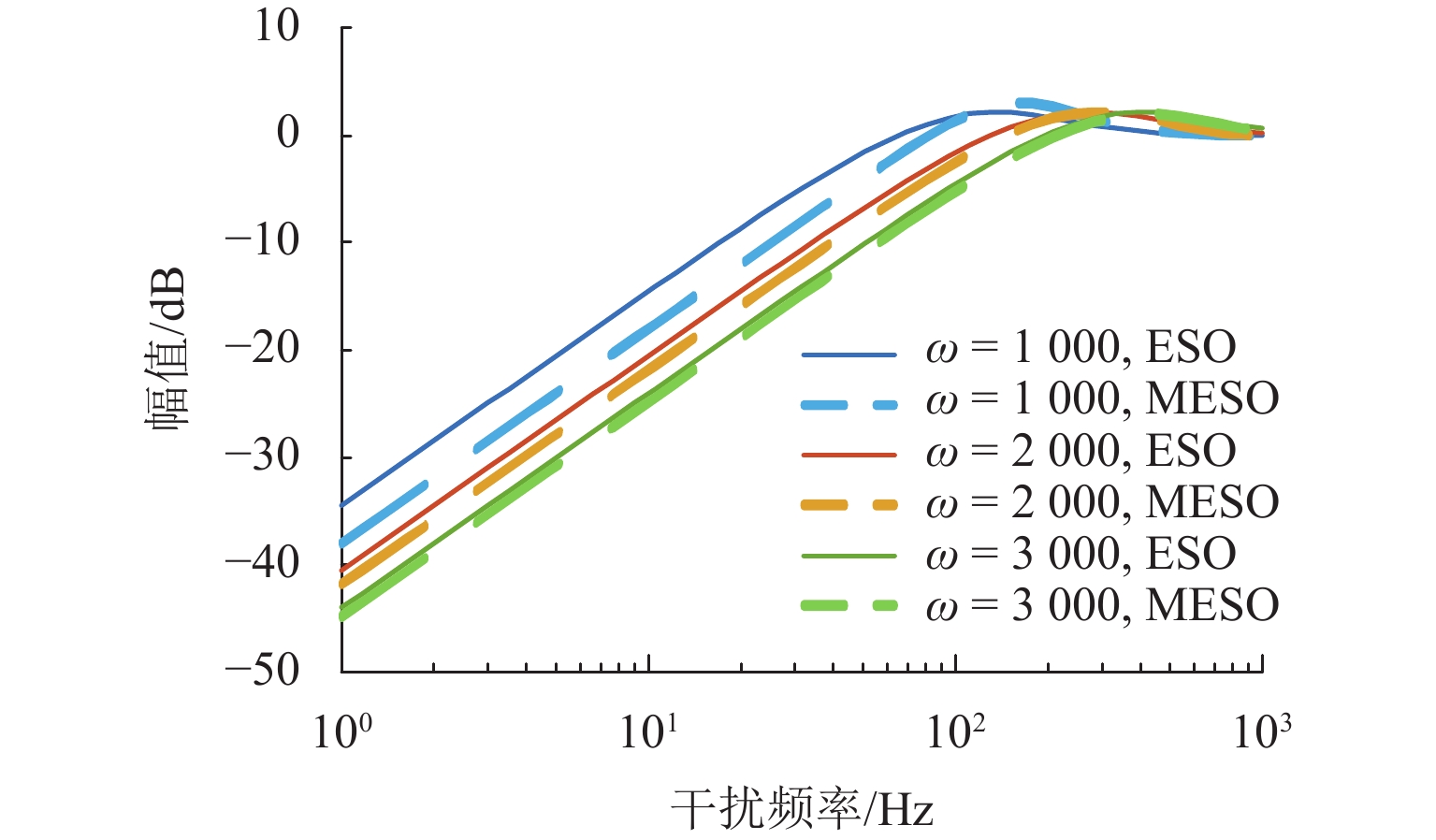
H2(s)=−[s3+3ωs2+(3ω2+kxm)s]s3+3ωs2+(3ω2+kxm)s+ω3+3ωkxm. (19) H2(s)的伯德图如图3所示. 从图中可以看出:带宽越大,MESO的效果逐渐与ESO相近,因为带宽越大式(17)中的模型信息对于带宽的影响就越小;因为噪声的存在,系统的带宽是有限的,所以MESO在实际工作条件下会具有更好的干扰抑制效果. 此外,不管是ESO还是MESO,对于高频正弦干扰的抑制效果都很差,但是,MESO能够采用更小的带宽在工作频率内实现更好的干扰抑制效果.
3. 稳定性分析
3.1 PD控制器设计
ADRC中ESO负责抑制系统受到的干扰,PID控制器则保证系统的闭环性能. ESO将系统控制电压以外的量扩张为新的状态变量并抵消,因此,系统被简化成串联积分器的形式,只需PD控制器即可保证系统的稳定[16]. 对于AMB转子系统来说,比例系数kp按照自然刚度的选取原则[1],即kp=2kx/(kikaks),此时系统闭环等效支撑刚度与AMB位移刚度基本相同;微分系数kd按照自然阻尼的原则选取,即kd = 2ξ √mkx/(kikaks),其中,ξ为阻尼比,取值范围为0.1<ξ<1.0.
3.2 系统稳定性分析
本节对提出的控制算法进行稳定性证明,AMB转子系统参数如表1所示.
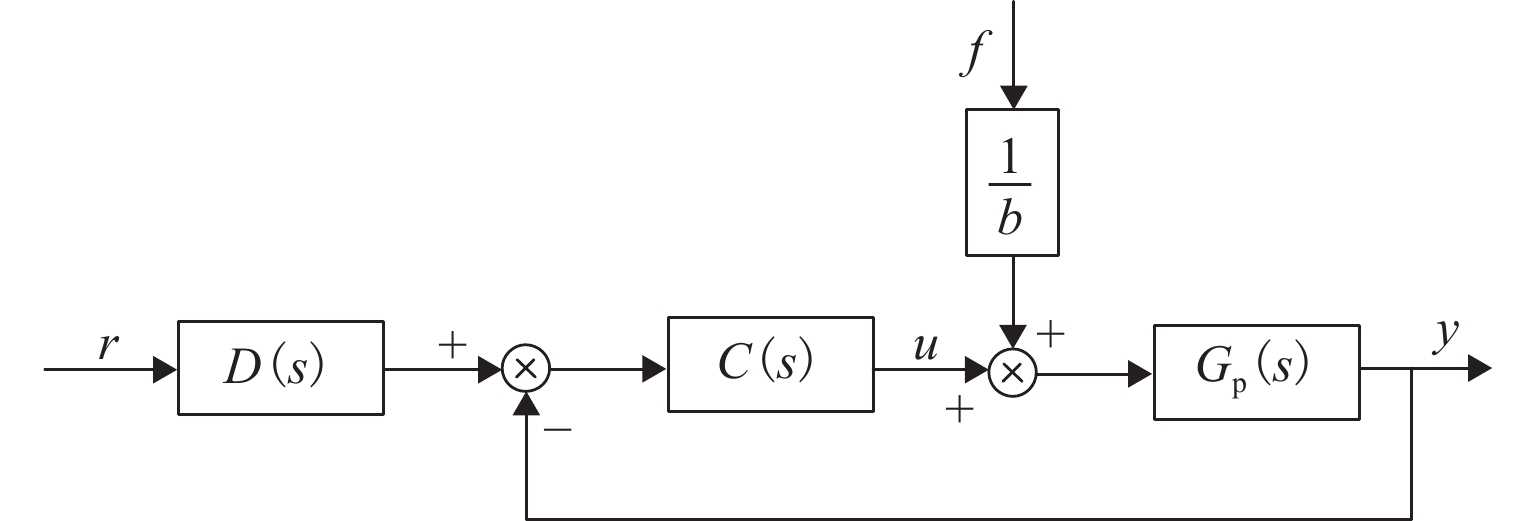
表 1 AMB转子系统参数Table 1. Parameters of AMB rotor system符号 参数值 m/kg 3.55 A/m2 2 × 10−4 N/匝 150 g0/mm 0.2 保护轴承单边间隙 gmin/mm 0.125 I0/A 1.3 α/(°) 22.5 μ0/(N·A−2) 4π × 10−7 ka/(A·V−1) 0.26 ks/(V·m−1) 20000 转子转速 n/(r·min−1) 3000 kp 1.8 kd 0.002 ω 3000 Jin等[17]提出一种ADRC标准框图,如图4所示,将ADRC等效为传递函数进而在频域内分析系统的稳定性. 图中:C(s)为系统的补偿器,对系统的外部干扰进行抵消; D(s)为输入参考的柔化器,减小系统的超调,对系统稳定性没有影响;Gp(s)为AMB系统数学模型;b为输入系数,b=kikaks/m.
因为采用PD控制,所以系统控制器的输出电压为
u=1b(kpr−kpz1−kdz2−z3). (20) 对于ADRC控制器来说,输入分别为y与r,输出只有u. 根据叠加原理可由式(15)、(20)求得u分别对y与r的传递函数,如式(21).
{U(s)Y(s)=(L1kp+L2kd+L3)s2+(L2kp+L3kd)s+kpL3−bs[s2+(L1+kd)s+L1kd+L2+kp],U(s)R(s)=kps3+L1kps2+L2kps+L3kpbs[s2+(L1+kd)s+L1kd+L2+kp], (21) 式中:U(s)、R(s)、Y(s)分别为u、r和y的拉普拉斯变换表达式.
令
{C(s)=−U(s)Y(s),D(s)=−Y(s)R(s)=kps3+L1kps2+L2kps+L3kp(L1kp+L2kd+L3)s2+(L2kp+L3kd)s+kpL3. (22) 根据式(22)可以得到系统的闭环传递函数为
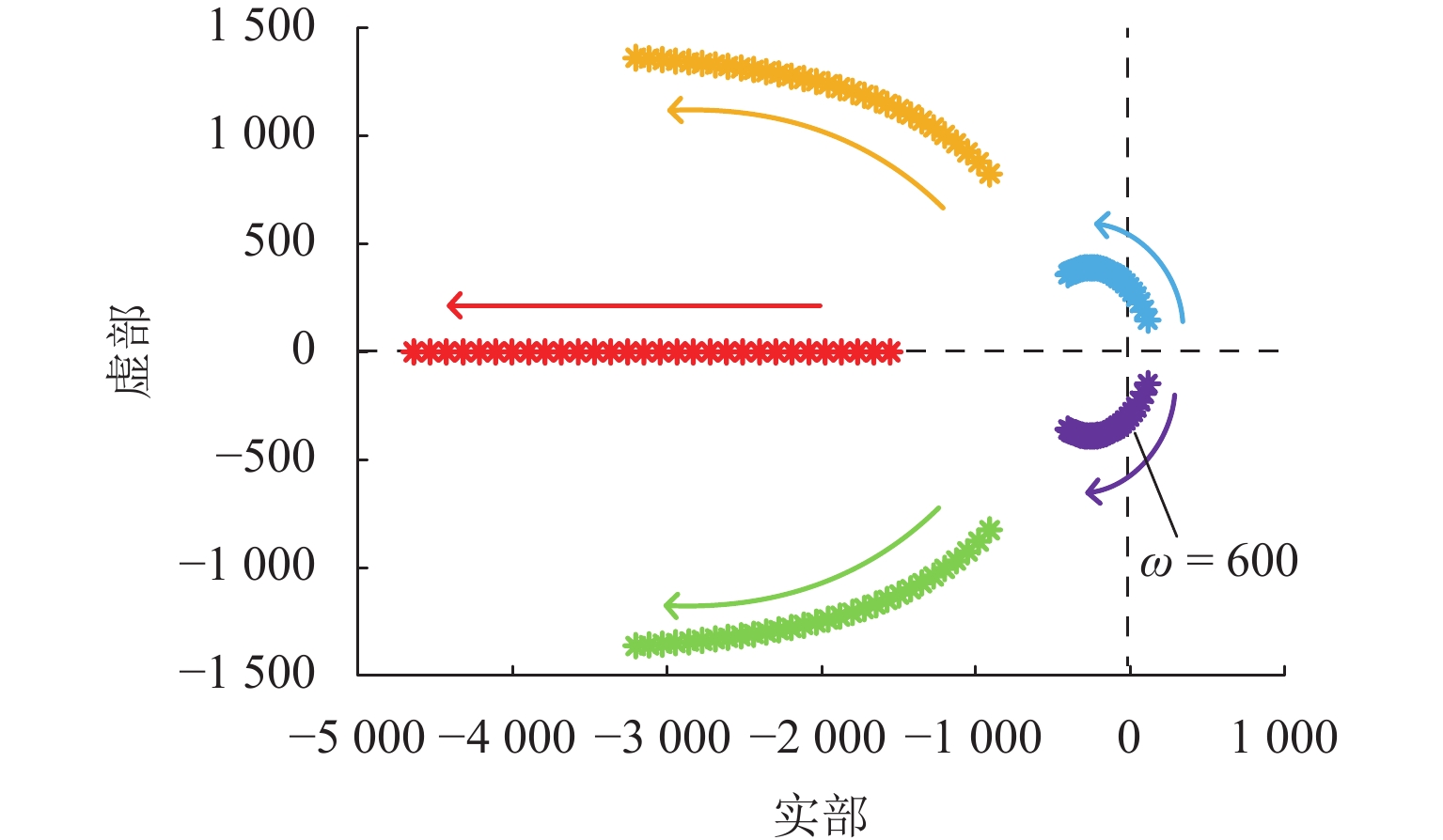
G(s)=C(s)Gp(s)1+C(s)Gp(s). (23) 当控制器的参数确定时,只有观测器带宽ω的大小会影响系统的稳定性. 绘制G(s)的特征方程根轨迹随ω从100~3000的变化趋势如图5所示. 从图中可以看出,只有当观测器带宽低于600时系统才会失稳,因为此时带宽过小,所以ESO无法准确地观测系统受到的广义扰动. 本文采用的带宽数值远大于600,所以系统是稳定的.
4. 仿真分析
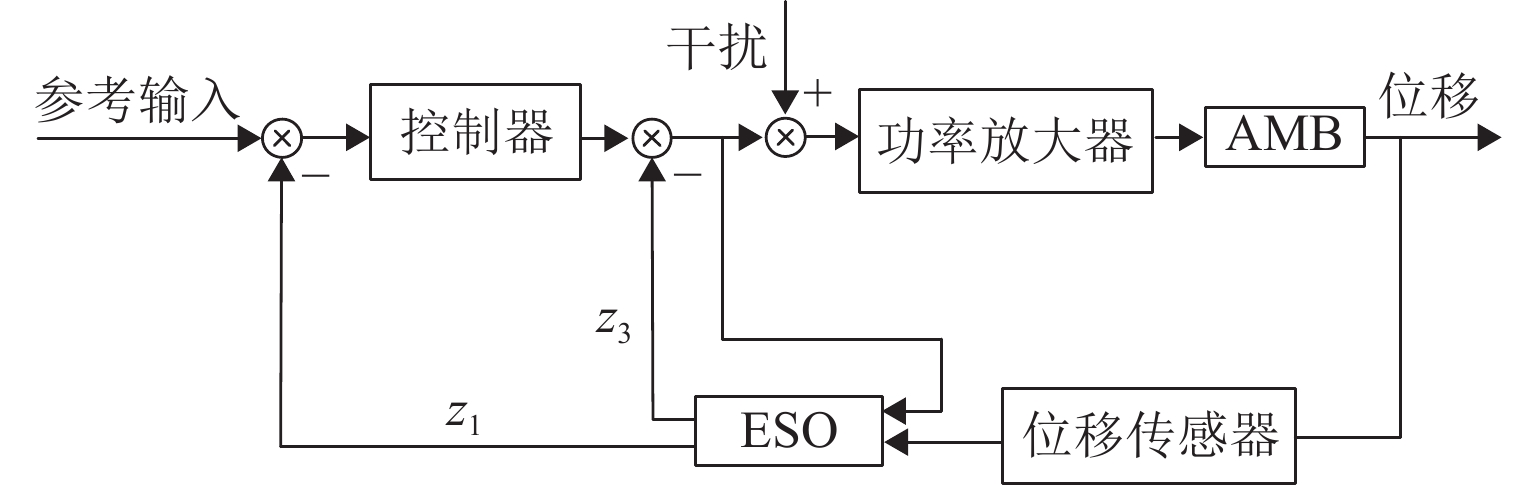
本节通过仿真验证提出算法的有效性,图6为ADRC控制框图,其中,ESO根据控制电压、转子位移计算出广义扰动z3与位移z1,最后在控制器处抵消系统所受的干扰,从而降低转子的振幅.
4.1 带宽的影响
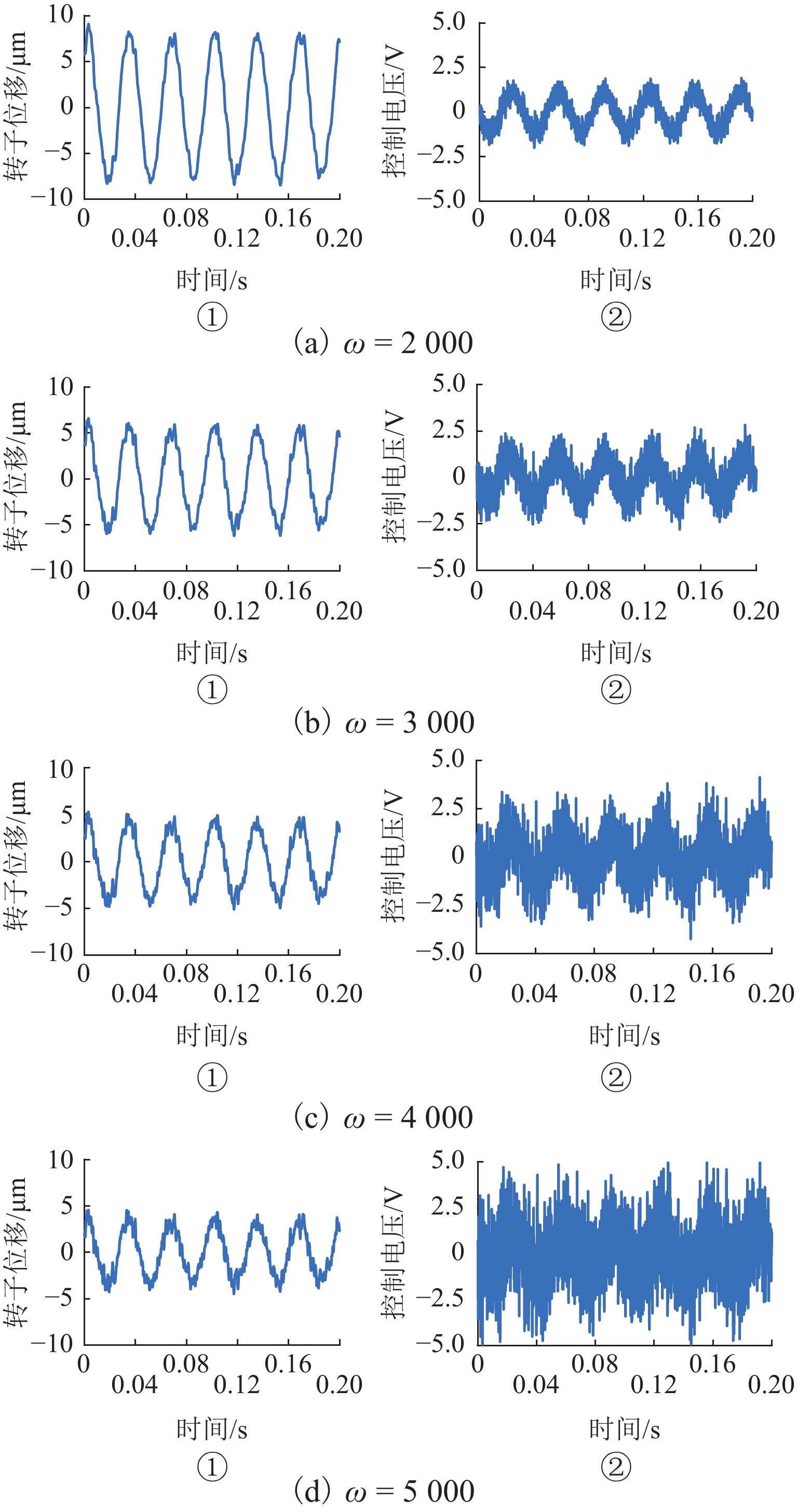
由2.1节可知,带宽ω越大,ESO对扰动的抑制效果就越好,但是,磁悬浮轴承系统在实际工作过程中不可避免地会受到噪声的影响,所以ESO的带宽并不能无上限地增加. 为了验证带宽的影响,对系统施加1 V和30 Hz的正弦干扰,假设系统受到的白噪声幅值为0.001,设置ESO的带宽ω=2000,3000,4000,5000,转子位移以及控制电压的仿真结果如图7所示. 可以看出,随着带宽ω的增大,ESO对扰动的抑制效果越好,ω=3000时转子的位移相对于ω=2000有较大的降低,当ω增加到4000时,噪声的存在使得系统控制电压波动剧烈并且正弦性变差;当ω=5000时,转子位移相对于ω=4000时提升很小,但是此时控制电压的波动十分剧烈,并且超出了限幅范围. 因此,实际过程中,ESO带宽的选择需要合理平衡扰动抑制效果以及噪声的影响.
4.2 阶跃干扰下转子位移仿真
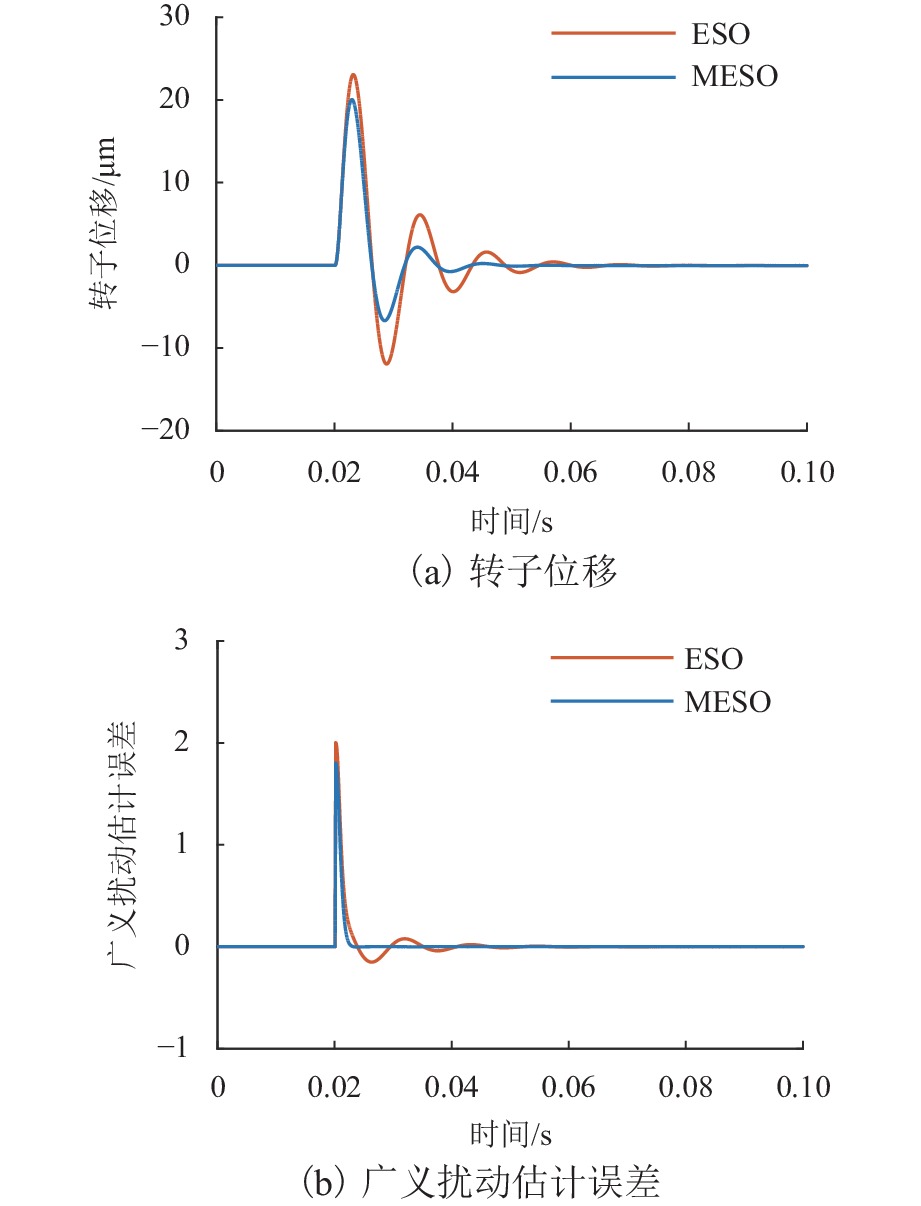
为了验证MESO抗阶跃干扰的性能,在0.02 s时对系统施加2 V的阶跃干扰. 图8(a)为不同控制器下的转子位移,可以看出,ESO下转子位移峰值为23 μm,MESO下转子位移峰值为20 μm,与ESO相比降低了13%,并且具有更快的收敛速度. 根据式(6)可知,系统的广义扰动分为系统的负位移刚度项kxx2/m与外部干扰项f/m两部分. MESO在干扰估计回路中给了实际的kxx2/m,降低了ESO的干扰估计量,提高了估计效果. 图8(b)为广义扰动估计值与实际值的误差,其中,MESO的广义扰动估计误差降低了约10%.
4.3 正弦干扰下转子位移仿真
为了验证MESO抗正弦干扰的性能,对不同控制器下的转子系统进行1 V、0~200 Hz的正弦信号扫频仿真,结果如图9所示. 从图中可以看出,相对于PID来说,观测器的引入可以有效降低外部干扰对转子位移的影响,但是也使得转子的刚体模态从60 Hz推迟到了90 Hz. MESO比ESO具有更好的干扰抑制效果,且能够更好地抑制转子刚体模态所引起的振动. 但是当干扰频率高于100 Hz时,ESO与MESO的干扰抑制效果不断下降并逐渐趋近于0,与第2节中分析的结果一致.
5. 试验验证
图10为基础激励下磁悬浮转子系统试验平台现场图. 转子由两个径向AMB与一个轴向AMB支承,通过变频器驱动转子旋转. 振动控制系统用来控制振动台对AMB转子系统施加基础振动,电脑通过dSPACE对磁悬浮转子试验台进行控制. 其中,系统的控制器都为PID控制器,参数如表1所示,观测器分别为ESO与MESO,对系统噪声与观测效果进行权衡后设置带宽ω=2000. 本文针对五自由度磁悬浮转子进行悬浮与旋转实验,由于磁悬浮转子实验台径向自由度采用的是分散控制,因此,只针对径向第一自由度的试验结果进行说明,其他3个径向自由度的试验结果均与径向第一自由度试验结果相类似.
5.1 静态悬浮抗干扰试验
5.1.1 阶跃扰动试验
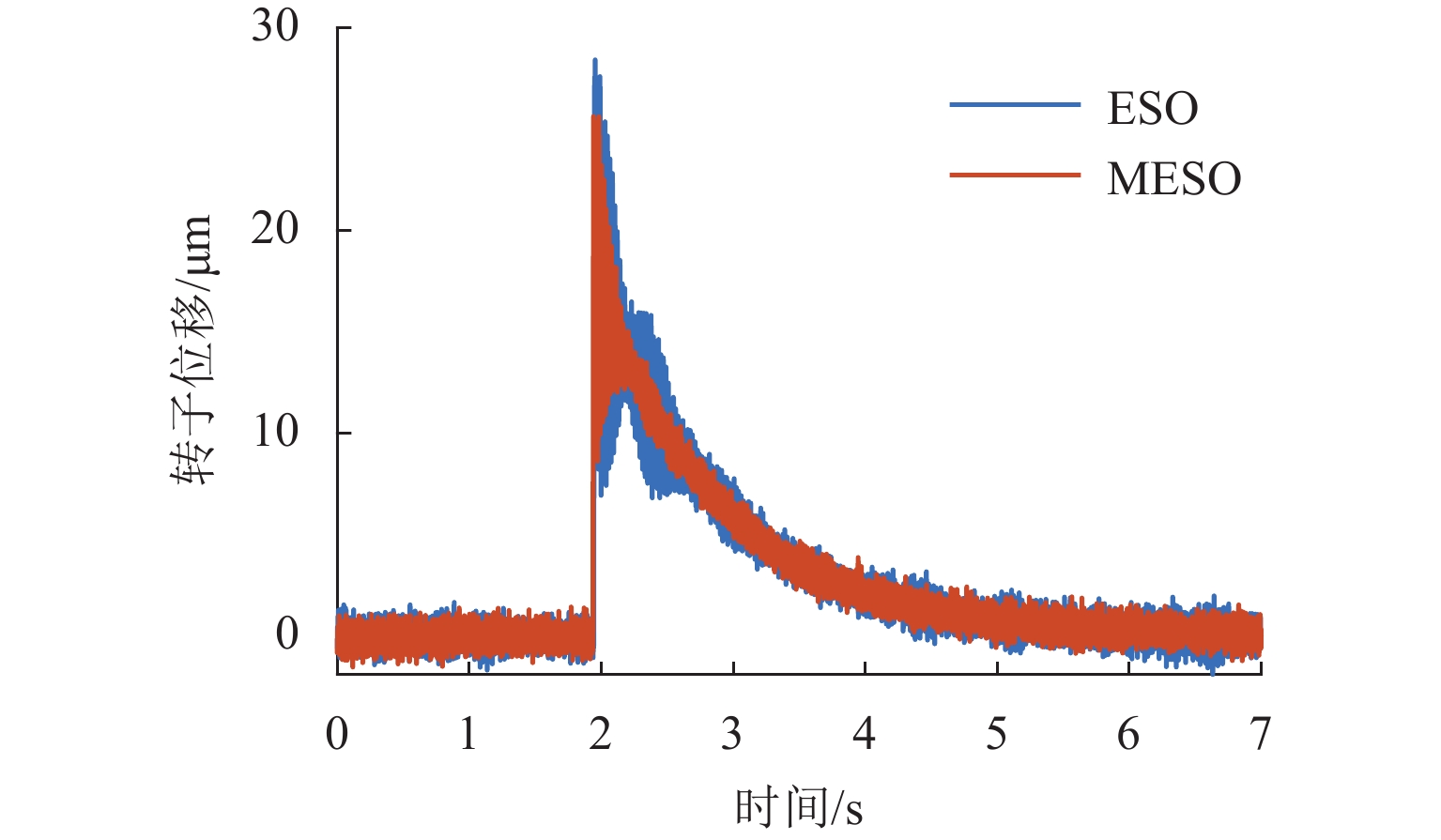
在转子静态悬浮时,对其施加0.5 V的阶跃干扰,通过对比转子振幅的变化衡量不同控制器的效果. 图11为阶跃干扰下转子的位移. 可以看出,阶跃干扰下,ESO、MESO的位移峰值分别为28.6、25.4 μm,与ESO相比MESO降低了11.2%,并且其下降过程中波动更低. 说明在静态悬浮下,MESO可以有效抑制阶跃干扰.
5.1.2 正弦扰动试验
为了验证控制器对不同频率正弦干扰的抑制效果,转子静态悬浮时对一路施加0~200 Hz不断变化正弦干扰. 转子的位移如图12所示,图中转子位移的两个峰值分别为转子的刚体模态与一阶弯曲模态. 可以看出,相对于PID控制,观测器的引入使得转子的刚体模态后移了大约10 Hz,但是观测器明显降低了转子模态处的最大峰值,与前文仿真部分的结论相一致.
尽管随着干扰频率的提高,观测器的效果会不断下降,但是观测器对于转子的模态振幅有明显的抑制作用. 图12中可以明显看出在整个转速频率内,MESO比ESO都具有更好的干扰抑制效果. 为了具体衡量控制器的效果,采用二范数对转子的位移进行计算,具体的数值如表2所示. 表中,ePID、eESO2、eMESO分别为PID、PID+ESO、PID+MESO控制器下0~200 Hz扫频转子位移. 可以看出MESO的整体效果比ESO高约15%.
表 2 转子位移二范数Table 2. 2-Norm of rotor displacement名称 二范数大小 相比 PID 控制器振幅降低比例/% ||ePID||2 7261.6 ||eESO||2 5684.6 21.7 ||eMESO||2 4608.7 36.5 5.2 基础简谐激励下磁悬浮转子抗干扰试验
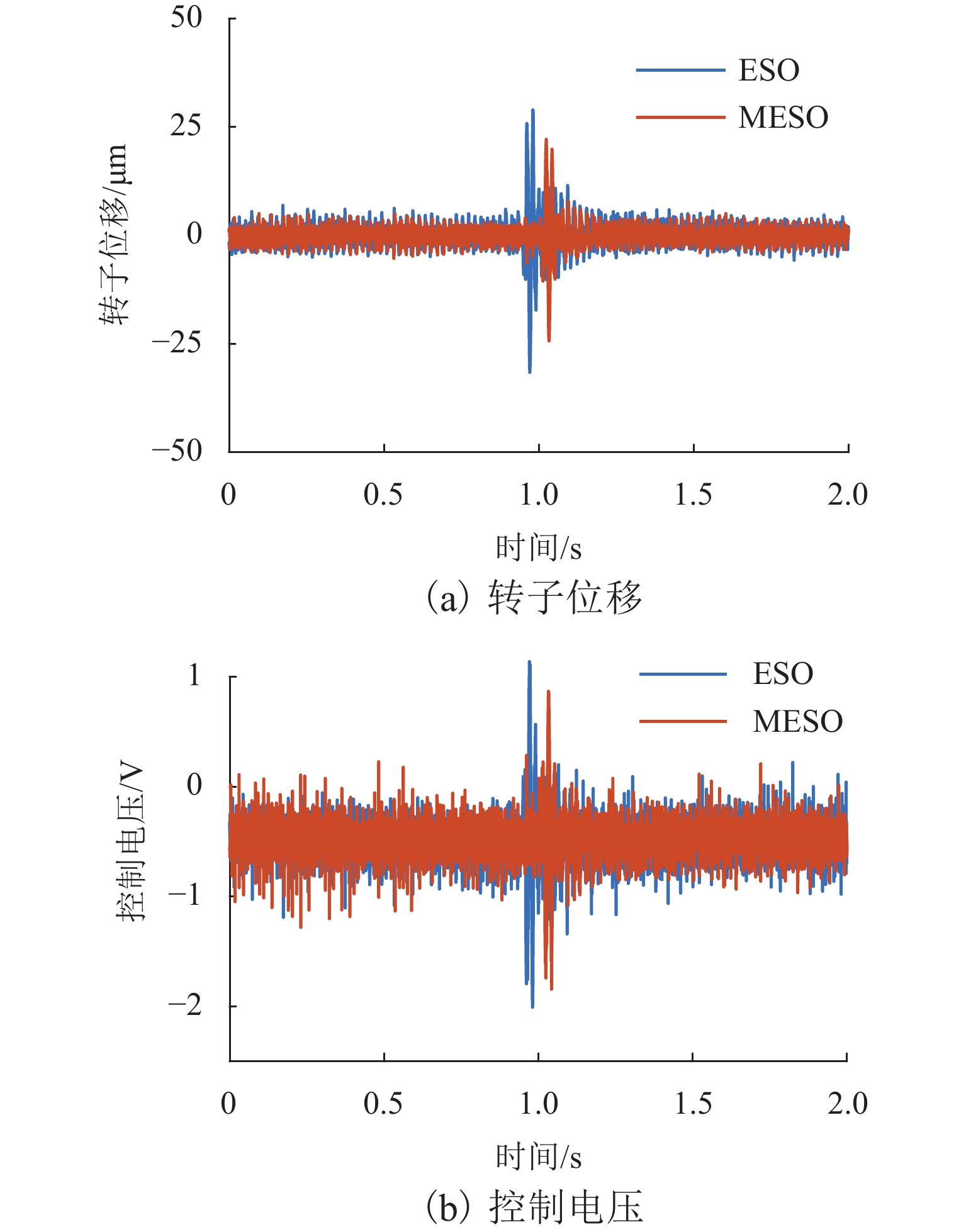
为验证所提出方法在转子旋转时抑制简谐激励的效果,在50 Hz旋转频率下加入频率为10 Hz,幅值为2 mm的基础振动作为外部干扰,分别比较转子位移与控制电压的大小,如图13. 图13(a)中ESO的位移峰峰值为36.2 μm,MESO的位移峰峰值为30.3 μm,降低了16.3%;图13(b)中,MESO下控制器输出的控制电压峰峰值为1.61 V,与ESO的1.85 V相比,降低了约13%. 说明对于基础振动产生的低频干扰,MESO可以用更小的控制电压实现更好的干扰抑制效果.
5.3 基础冲击激励下磁悬浮转子抗干扰试验
为了验证所提出方法在转子旋转时抑制冲击激励的效果,在50 Hz旋转频率下施加幅值为1的冲击干扰,试验中转子的位移响应与控制电压如图14所示. 图14(a)中,ESO的位移峰峰值为59.7 μm,MESO的位移峰峰值为46.2 μm,相比ESO降低了22.6%;图14(b)中,MESO的控制电压峰峰值为2.71 V,相比ESO的3.15 V降低了14.0%. 因此,对于冲击干扰,所提出的MESO也可以用更小的控制电压实现更好的控制效果.
6. 结 论
为了提高ESO的干扰抑制效果,本文提出了一种模型辅助扩张状态观测器,并在频域内分析了控制系统的稳定性. 通过仿真与试验验证了所提出方法的有效性,得出以下结论:
1) 带宽越大,ESO对干扰的估计效果越好. 但是带宽的增加会放大噪声的影响,使得系统的控制电压产生剧烈波动.
2) 随着干扰频率的提高,ESO与MESO的干扰估计效果都会逐渐下降,但是仍然可以对转子模态振幅进行有效抑制.
3) 与ESO相比,本文提出的MESO在相同的带宽下具有更大的观测器增益,因此,在工作频率内相比ESO具有更好的干扰抑制效果.
4) 对50 Hz旋转频率下的转子分别施加10 Hz、2 mm的基础简谐干扰与1g的基础冲击干扰,试验结果表明,MESO控制下的转子位移相比ESO分别降低了16.3%与22.6%,控制电压比ESO降低了14.0%左右,说明MESO可以用更小的控制电压实现更优的控制效果.
-
表 1 AMB转子系统参数
Table 1. Parameters of AMB rotor system
符号 参数值 m/kg 3.55 A/m2 2 × 10−4 N/匝 150 g0/mm 0.2 保护轴承单边间隙 gmin/mm 0.125 I0/A 1.3 α/(°) 22.5 μ0/(N·A−2) 4π × 10−7 ka/(A·V−1) 0.26 ks/(V·m−1) 20000 转子转速 n/(r·min−1) 3000 kp 1.8 kd 0.002 ω 3000 表 2 转子位移二范数
Table 2. 2-Norm of rotor displacement
名称 二范数大小 相比 PID 控制器振幅降低比例/% ||ePID||2 7261.6 ||eESO||2 5684.6 21.7 ||eMESO||2 4608.7 36.5 -
[1] MASLEN E H, SCHWEITZER G. Magnetic bearings: theory, design, and application to rotating machinery[M]. Heidelberg: Springer, 2009. [2] 刘淑琴, 边忠国, 李瑞建, 等. 磁悬浮人工心脏泵及在体外循环系统(ECMO)上的实验研究[J/OL]. 西南交通大学学报. [2022-12-10]. http://kns.cnki.net/kcms/detail/51.1277.U.20220426.1933.010.html. [3] DU G H, XU W, ZHU J G, et al. Effects of design parameters on the multiphysics performance of high-speed permanent magnet machines[J]. IEEE Transactions on Industrial Electronics, 2020, 67(5): 3472-3483. doi: 10.1109/TIE.2019.2922933 [4] 钟志贤,蔡忠侯,祁雁英. 单自由度磁悬浮系统无模型自适应控制的研究[J]. 西南交通大学学报,2022,57(3): 549-557,581.ZHONG Zhixian, CAI Zhonghou, QI Yanying. Model-free adaptive control for single-degree-of-freedom magnetically levitated system[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 549-557,581. [5] ZHANG S Y, WEI C B, LI J, et al. Robust H∞ controller based on multi-objective genetic algorithms for active magnetic bearing applied to cryogenic centrifugal compressor[C]//Proceedings of the 29th Chinese Control And Decision Conference. Chongqing: IEEE, 2017: 46-51. [6] XU Y P, SHEN Q, ZHANG Y, et al. Dynamic modeling of the active magnetic bearing system operating in base motion condition[J]. IEEE Access, 2020, 8: 166003-166013. doi: 10.1109/ACCESS.2020.3022996 [7] XU X B, FANG J C, LIU G, et al. Model development and harmonic current reduction in active magnetic bearing systems with rotor imbalance and sensor runout[J]. Journal of Vibration and Control, 2015, 21(13): 2520-2535. doi: 10.1177/1077546313513624 [8] KATO J, TAKAGI K, INOUE T. On the stability analysis of active magnetic bearing with parametric uncertainty and position tracking control[C]// Proceedings of ASME 2016 International Mechanical Engineering Congress and Exposition. Phoenix: [s.n.], 2017: 1-10 [9] HAN J Q. The extended state observer of a class of uncertain systems[J]. Control and Decision, 1995, 10(1): 85-88. [10] JIN C W, GUO K X, XU Y P, et al. Design of magnetic bearing control system based on active disturbance rejection theory[J]. Journal of Vibration and Acoustics, 2019, 141(1): 011009.1-011009.9. doi: 10.1115/1.4040837 [11] GUAN X D, ZHOU J, JIN C W, et al. Disturbance suppression in active magnetic bearings with adaptive control and extended state observer[J]. Proceedings of the Institution of Mechanical Engineers, Part I: Journal of Systems and Control Engineering, 2020, 234(2): 272-284. [12] GUO B Z, ZHAO Z L. On the convergence of an extended state observer for nonlinear systems with uncertainty[J]. Systems & Control Letters, 2011, 60(6): 420-430. [13] ZHENG Q, GAOL L Q, GAO Z Q. On stability analysis of active disturbance rejection control for nonlinear time-varying plants with unknown dynamics[C]//2007 46th IEEE Conference on Decision and Control. Louisiana: IEEE, 2008: 3501-3506. [14] SUN B S, GAO Z Q. A DSP-based active disturbance rejection control design for a 1-kW H-bridge DC-DC power converter[J]. IEEE Transactions on Industrial Electronics, 2005, 52(5): 1271-1277. doi: 10.1109/TIE.2005.855679 [15] GODBOLE A A, KOLHE J P, TALOLE S E. Performance analysis of generalized extended state observer in tackling sinusoidal disturbances[J]. IEEE Transactions on Control Systems Technology, 2013, 21(6): 2212-2223. doi: 10.1109/TCST.2012.2231512 [16] GAO Z Q. Scaling and bandwidth-parameterization based controller tuning[C]//Proceedings of the 2003 American Control Conference. Ohio: IEEE, 2003: 4989-4996. [17] JIN H Y, SONG J C, ZENG S, et al. Linear active disturbance rejection control tuning approach guarantees stability margin[C]//2018 15th International Conference on Control, Automation, Robotics and Vision (ICARCV). Singapore: IEEE, 2018: 1132-1136 期刊类型引用(4)
1. 王麒臻,张俊梅,徐向波. 基于自适应陷波器与ESO的磁悬浮转子振动抑制方法. 微特电机. 2025(02): 52-59 .  百度学术
百度学术2. 黄刚,袁崧琳,于惠钧,时鑫一,刘博龙. 基于等价输入干扰滑模观测器的磁悬浮轴承控制. 轴承. 2024(07): 80-86+94 .  百度学术
百度学术3. 王浩,龙志强,丁明强,王屹华. 基于改进模型辅助ESO的磁轴承-转子系统自抗扰控制. 轴承. 2024(07): 132-137 .  百度学术
百度学术4. 巩磊,何派,石勇,祝长生. 主动磁悬浮轴承有限元模型转子位置反步法控制. 陕西科技大学学报. 2024(06): 157-164 .  百度学术
百度学术其他类型引用(3)
-






 下载:
下载:













 下载:
下载:




 百度学术
百度学术
