Self-Learning Model Reference Adaptive Levitation Control Strategy
-
摘要:
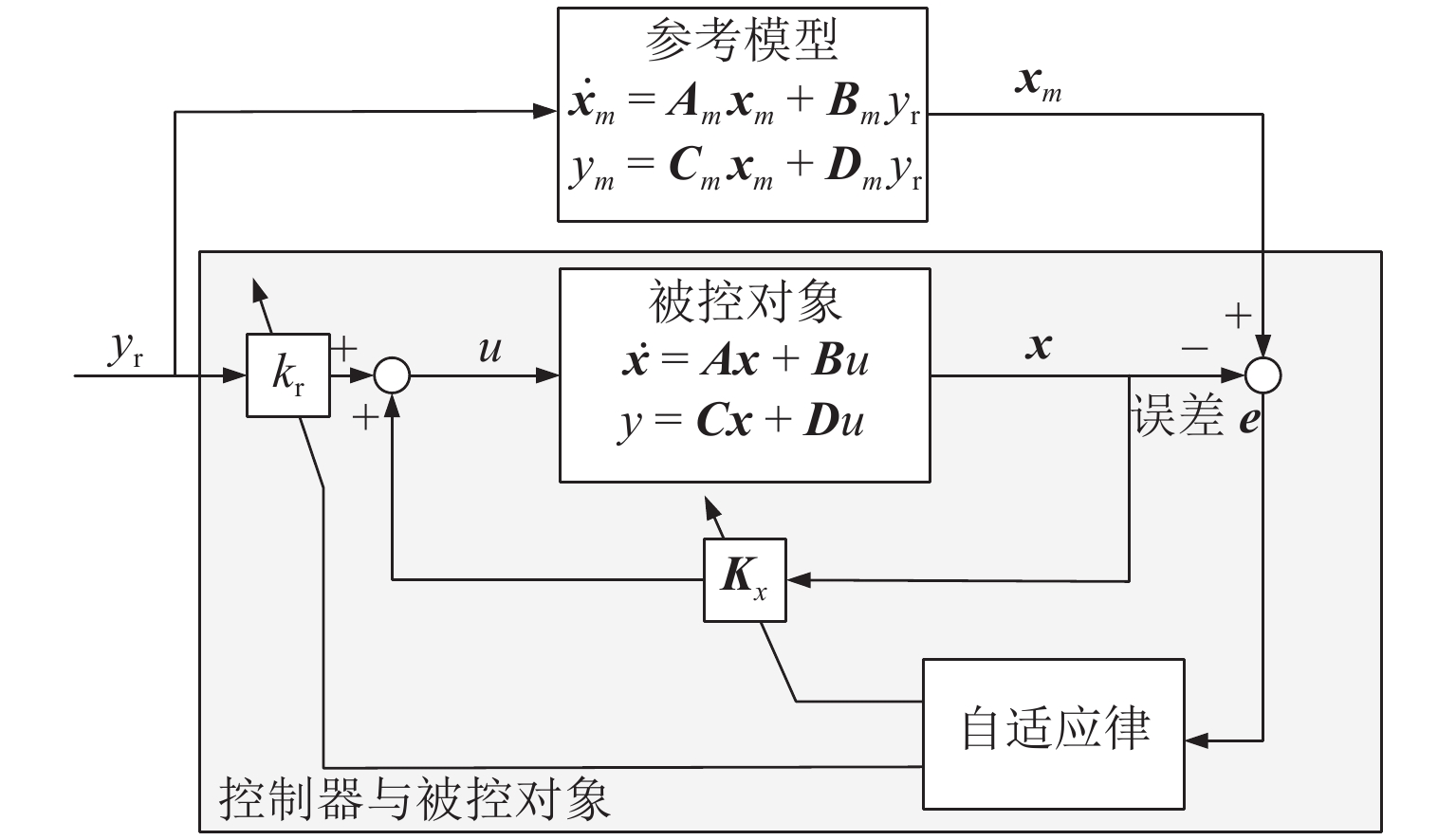
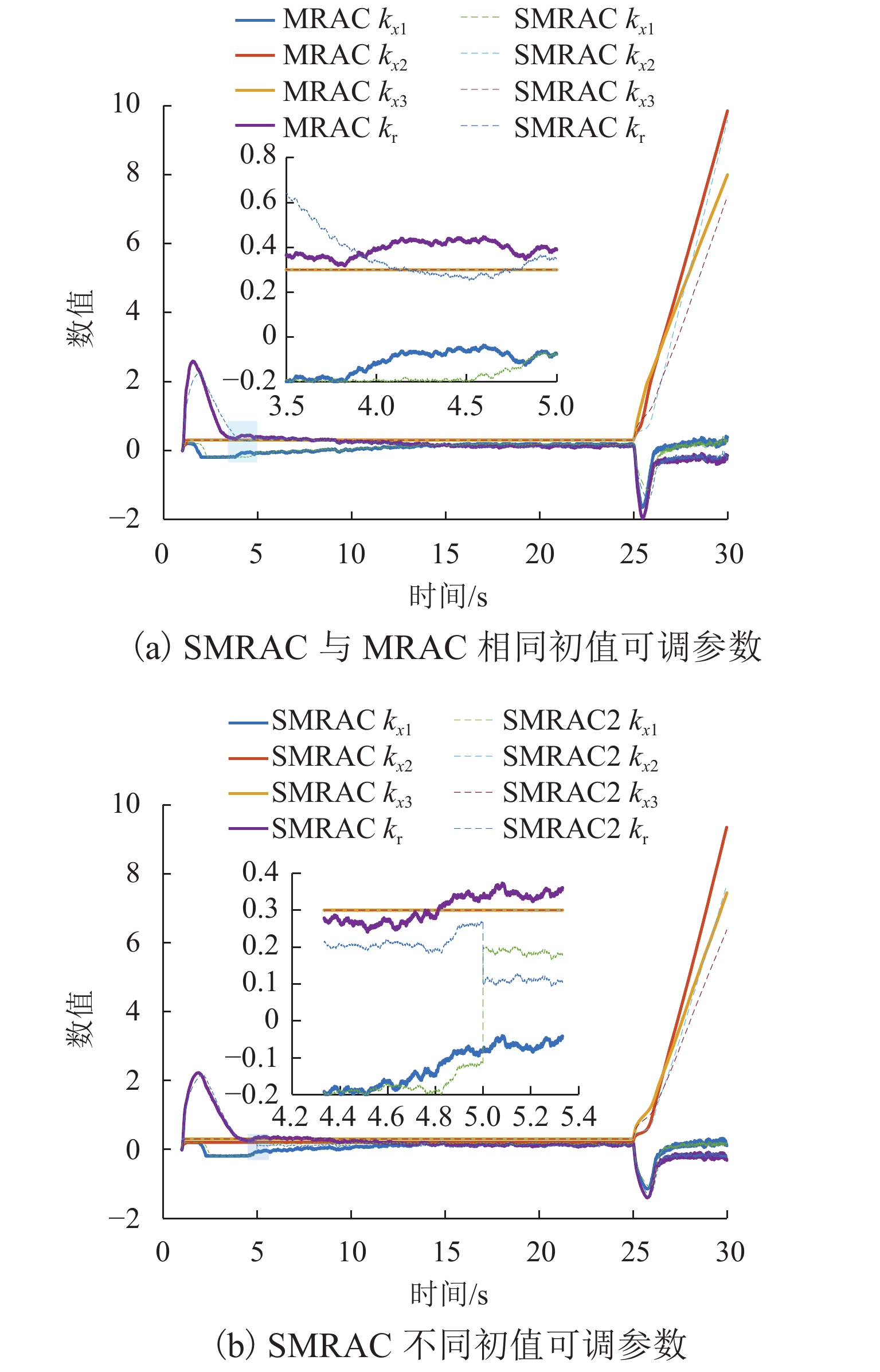
针对电磁悬浮列车悬浮控制器因轨道不平顺所引发的未知非线性力和传递函数不确定问题,提出一种基于模型参考自适应的自学习控制方案,控制算法中可调参数根据系统状态、误差和时间调整,使悬浮间隙稳定在恒定数值;学习率根据目标间隙误差大小动态调节,避免可调参数调节过慢,同时保证在稳定悬浮时间隙波动更小;通过李雅普诺夫稳定性判据证明了模型参考自适应控制系统的稳定性;通过MATLAB/Simulink对所提出的控制方案进行仿真. 研究结果表明:自学习模型参考自适应控制算法间隙的均方根误差为0.12,设定合适的可调参数初始值并对其限幅能够提升控制器的鲁棒性;在单悬浮架测试时,控制器获取到加速度信号,所提出算法的上升时间和调节时间分别为1.21 s和2.04 s,该方法学习率可动态调节,提升了控制器的适应能力.
Abstract:A self-learning model reference adaptive control strategy was proposed to solve the problems of unknown nonlinear force and uncertain transfer function of levitation controllers, which were caused by track irregularity in electromagnetic levitation trains. The tunable parameters in the control algorithm were adjusted according to the system state, error, and time, so as to make the gap stabilize at a constant value. In order to avoid slow adjustment of tunable parameters, the learning rate was dynamically adjusted according to the error of target gaps, so as to guarantee that the gap fluctuation was smaller during stable levitation. The stability of the model reference adaptive control system was confirmed by a Lyapunov framework, and the proposed control strategy was simulated by MATLAB/Simulink. The results show that the root-mean-square error (RMSE) of the gap of the self-learning model reference adaptive control algorithm is 0.12, and setting appropriate initial values of tunable parameters and limiting their amplitude can improve the robustness of the controller. When the algorithm is tested on a single levitation frame, the acceleration signal is obtained by the controller. The rising and adjustment time of the proposed algorithm is 1.21 s and 2.04 s, respectively. It proves that the learning rate of the method can be adjusted dynamically, which improves the adaptive ability of the controller.
-
Key words:
- maglev trains /
- levitation control /
- model reference adaptive /
- Lyapunov functions
-
据《“十四五”现代综合交通运输体系发展规划》报告[1],至2025年底我国高铁里程将达到5万公里. 铁路站场接触网是保障列车正常运行的重要设施,是铁路运维部门的重点巡查对象,保持接触网导线几何特征的正常状态,对铁路运输安全具有重要意义[2-5]. 高效的站场接触网导线特征检测为维持接触网正常运作提供保障,是智能铁路建设的重要一环.
站场接触网导线几何特征主要包括拉出值和导线高度值. 当前,接触网导线点云特征提取方法分为接触式和非接触式. 传统的人工点对点接触式检测方法存在安全风险大、占用天窗久,测量效率低、误差大等问题. 三维激光扫描技术为检测接触网导线几何特征提供了一种新思路[6-9]. 目前的研究主要集中在长距离铁路接触网导线特征提取,其严重依赖轨道车及接触网设备编号等,增加了铁路工人实际使用的难度,测量结果也存在较大误差. 而地面激光扫描精度高,满足站场场景近距离精确扫描要求. 通过从三维点云中提取接触网导线和钢轨顶面的点云数据,准确地分析和量化二者的相对位置关系,有效提取导线的几何特征. 接触网导线点云提取,一般采用随机采样一致性(random sample consensus, RANSAC)算法与3D Hough算法进行直线检测[10-11],无法很好地捕捉接触网导线弯曲的几何形状;对于复杂电力导线,采用基于半径邻域的空间维度特征和方向特征等提取电力线点[12],或是通过邻域半径自主选取、动态邻域特征进行点云提取分析[13-15],但对接触网复杂场景适应能力不足,存在过提取现象. 钢轨区域主要包括钢轨、轨枕和扣件,一般需要借助点云强度信息、高程信息或高分辨率影像匹配实现钢轨的提取[16-18],该方法对点云数据有质量要求,提取精度不高,无法适应复杂地区(如铁路站场)的铁路轨道精确提取.
针对复杂铁路站场接触网点云数据噪声无规律分布、语义分割难度大等问题[19-20],提出知识引导的站场接触网点云导线特征智能提取方法,通过厘清站场场景自身结构特征的分布规律,融合并改进多种滤波算法对不同特征的地面激光点云进行去噪与抽稀处理,并基于站场接触网严格设计规范,构建站场接触网强空间语义约束规则,建立知识引导的站场接触网点云导线与钢轨精细化智能提取方法,实现站场接触网导线几何特征智能检测.
1. 站场接触网点云导线特征检测方法
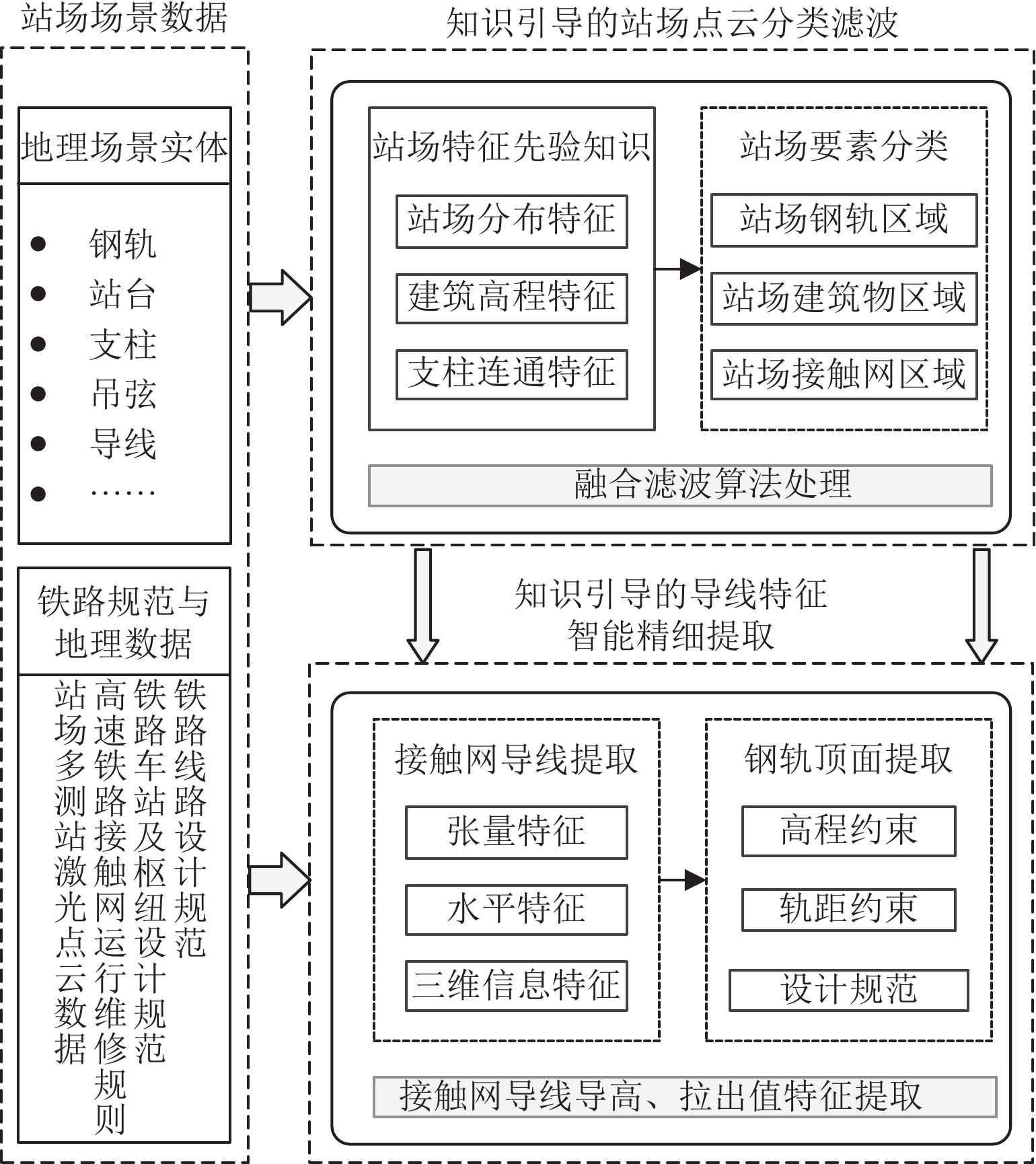
本文针对站场接触网场景进行研究,站台及钢轨成近似直线分布,具有广泛的通用性. 通过滤波获得低密度存储的高精度接触网点云,进而提取接触网导线点云与钢轨点云,通过欧式距离最近匹配算法进行接触网特征提取. 本文站场接触网导线特征智能提取总体研究路线如图1所示.
1.1 知识引导的站场点云分类滤波
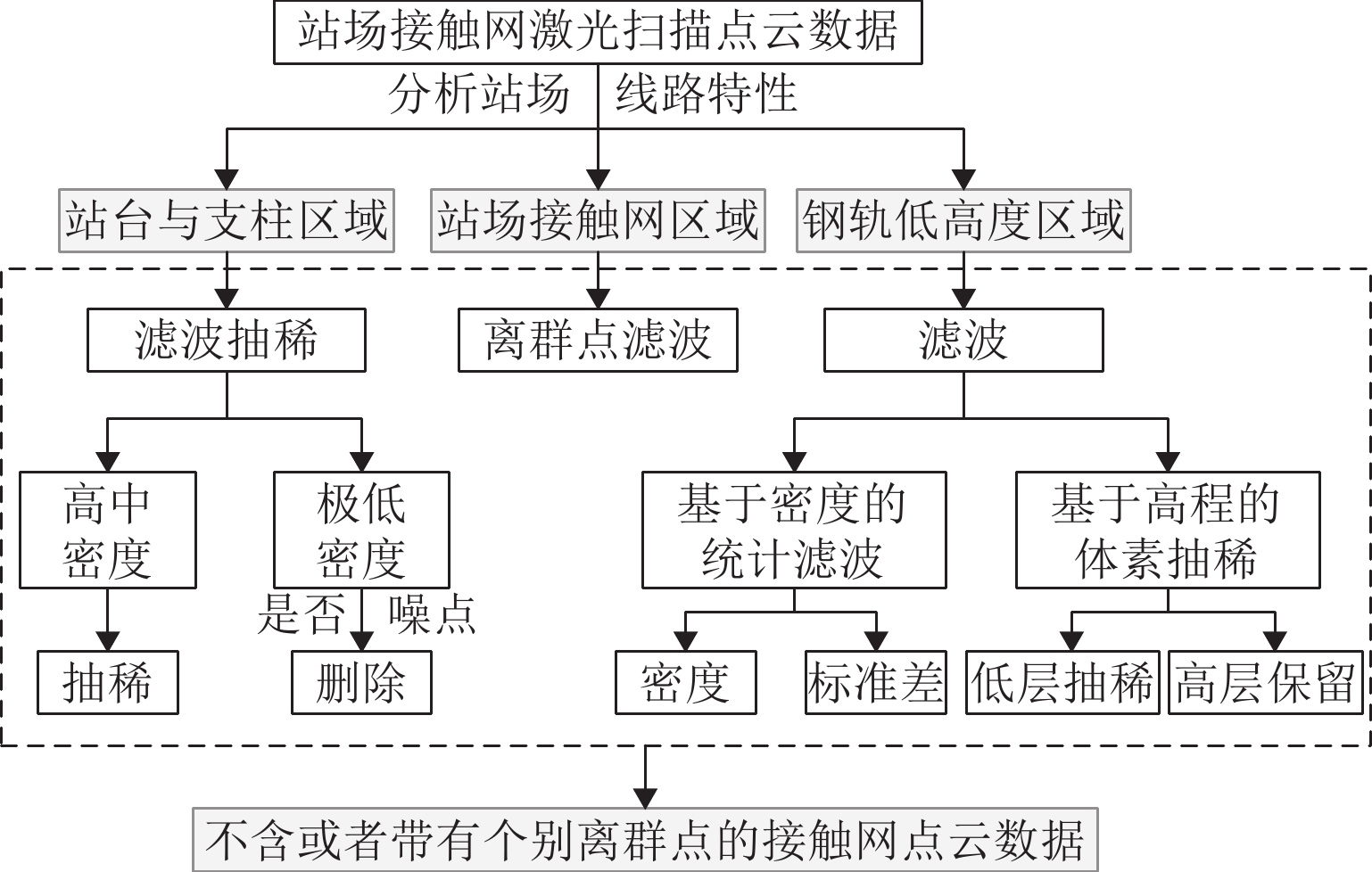
站场接触网点云数据中各电力要素的结构和密度存在差异,且要素周边噪声无明显规律[21-22]. 站场接触网点云具有明显的空间特征:1) 站场点云分布在水平面上呈带状直线分布;2) 接触网导线与支柱竖直维度上的连通性特征差异显著;3) 接触网导线成“之”字形水平分布与高度一致性特征;4) 站场铁路轨道设计严格,轨顶面存在高度一致性. 基于上述特征,提出一种知识引导的站场点云分类滤波方法,具体技术流程如图2所示.
1) 首先是点云分类,基于站场接触网的空间特征,将站场接触网点云划分为站场接触网区域、站场钢轨区域、站场建筑物区域.
根据站场支柱连通特征[23-24],在接触网与站场支柱建筑物连接位置,采用基于法向差异的滑窗分割算法将支柱与接触网分离. 具体过程为:首先,设置n行k列的竖直紧密排列的体素单元组成滑窗;然后,计算各个体素内点云数据指向接触网导线方向的平均法向量;接着,以滑窗中心体素为基准,计算其法向量与其他体素法向量的法线方向差异;最后,计算差异得分,第m个法向量的差异得分如式(1)所示.
wm=cosθmexp(dm), (1) dm=√3∑l=1|n0l−nml|2, (2) 式中:m∈[1,nk],l∈{1,2,3},θm为中心法向量n0与第m个法向量之间的夹角,dm为3个方向维度上的欧式距离权重函数,n0l为n0的第l个分量,nml为第i个法向量的第j个分量.
距离滑窗中心越远,di越大,该点为站场接触网导线区域的可能性越大.
2)针对站场钢轨点云,采用基于点云密度自适应的多层次统计滤波与基于高程的抽稀算法进行处理. 使用式(3)对点i的密度ρi进行计算.
ρi=1πr2N∑j=1I(||pi−pj||<r), (3) 式中:i, j∈[1, N],N为点云总点数;pi、pj分别为点云中点i、j的坐标;r为预定义的领域范围半径;I(||pi−pj||<r)表示当pi和pj之间的距离小于r,返回1,否则,返回0.
根据点云的密度情况,选择不同的统计滤波参数. 对于距离架设点距离较近的地方,适当增加统计滤波的邻域点个数,同时,根据噪声情况调整标准差乘数阈值. 基于高程的体素抽稀算法,对低层道床结构的高密度点云数据进行抽稀,保留高层钢轨结构的点云数据,进一步优化数据的结构和分布. 通过上述方法提高点云数据质量,降低后续计算复杂度.
3)针对站场建筑物点云,使用基于密度的多级抽稀方法进行处理. 在近处对密集的点云进行抽稀,保留中远处点云细节,有效去除离群点影响. 对于站场接触网点云,考虑到电力线点云数量较少,在同时保证导线点云数据量与质量情况下,采用半径滤波剔除离群点.
1.2 知识引导的导线特征智能精细提取
在铁路建造时,铁路站场与接触网建设与维护必须严格遵守《铁路线路设计规范》[25]、《铁路车站及枢纽设计规范》[26]与《高速铁路接触网运行维修规则》[27]等标准规范. 本文通过深入剖析复杂环境下站场接触网点云的结构特征,构建站场接触网强空间语义约束规则,建立基于知识引导的站场接触网点云导线精细化智能提取策略,实现站场接触网导线几何特征的快速准确提取,如图3所示.
1.2.1 站场接触网导线点云处理
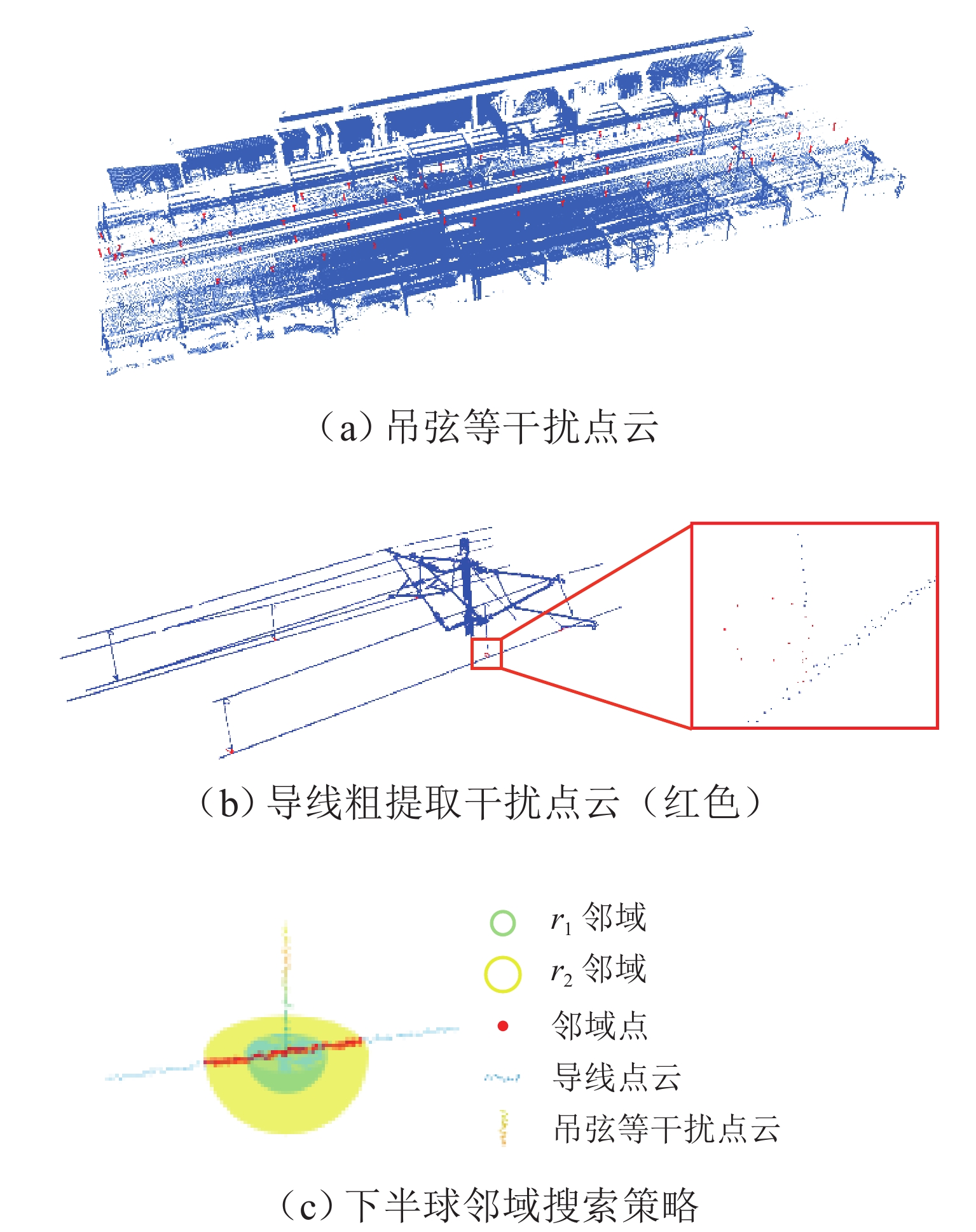
接触网导线场景复杂,除了接触网导线,还有吊弦、承力索、定位器、接触悬挂等干扰因素. 利用高程阈值分割,能有效降低非导线点的影响,可以减少大部分的非导线点.
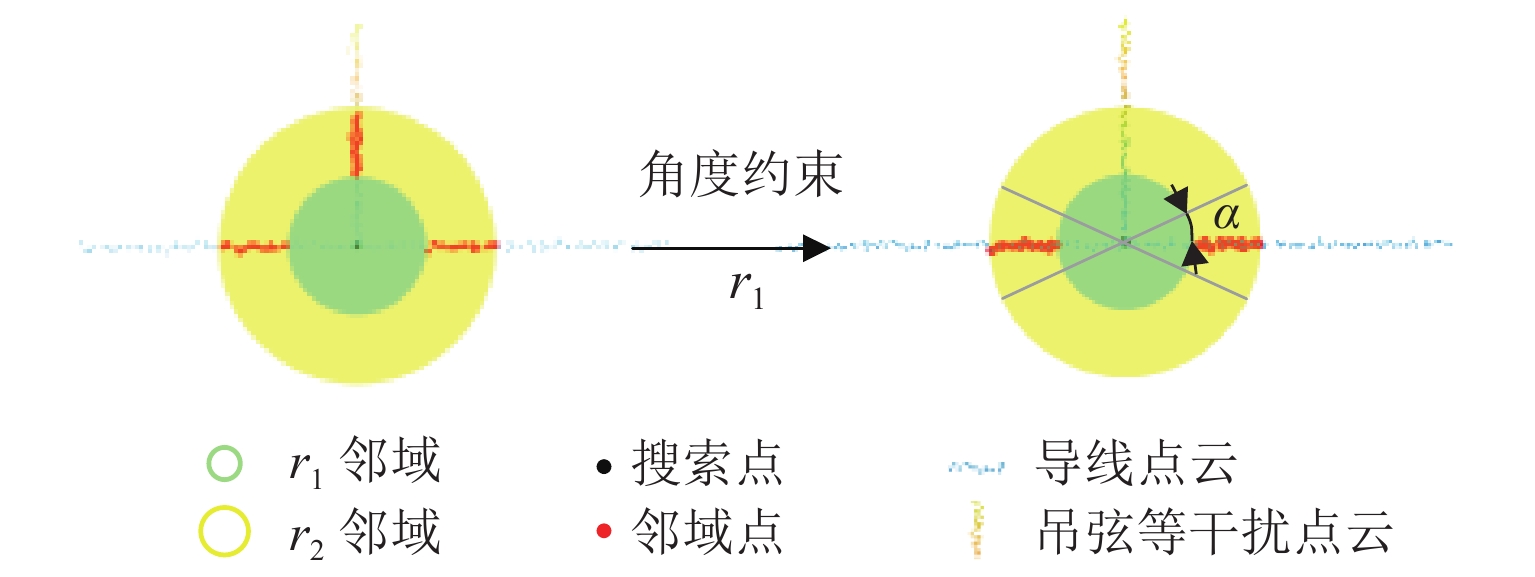
在此基础上,本文设计一种针对接触网导线的局部邻域特征点的自适应选择算法,计算接触网导线的空间分布几何特征. 在环形邻域基础上,进一步限制邻域点的角度范围,更精确地估计导线特征. 例如,规定正北方向为0°的坐标内,选择顺时针旋转70°~110°角度的点云作为该定位点的邻域范围. 以此来扩大所述接触网导线点云数据的线性特征,减少局部噪声与电力要素连接处的影响,从而更准确地反映站场接触网线状物体的几何特征. 邻域选择方法如图4所示,图中,r1、r2为不同的领域半径,α为规定的约束角度.
针对点云中某点i,通过图4方法选择邻域点,并计算其邻域的协方差矩阵Ci,如式(4)所示.
Ci=1JJ∑j=1(pij−¯pi)(pij−¯pi)T, (4) 式中: pij为点i的邻域点j的位置向量;J为邻域点的总数;¯pi为所有邻域点的平均位置,表示为¯pi=1JΣJj=1pij.
根据式(4)得到3个特征值λ1、λ2、λ3,且满足λ1>λ2>λ3. 结合导线点云的张量特征及水平特征,对分类后的接触网导线点云进行分割. 对于导线的线性特征,最大特征值是远大于其他2个特征值的,采用前2个特征值,构造如式(5)所示的站场接触网导线线性特征度量λL,通过设置适当阈值即可分割线性点云.
λL=λ1−λ2λ1. (5) 导线精细提取是去除与接触网导线相连的残余吊弦、接触悬挂等,如图5(a)、(b)所示. 借助接触网导线点云邻域高程差异进行水平导线分割,将差异较小的点视为水平方向上的点,即水平导线点. 通过干扰点云与导线位置的分析,干扰点云仅位于接触网导线的上方,使用下半球半径邻域搜索策略可以有效去除上方点云的干扰. 通过k-d树(k-dimensional tree)搜索邻域的点,计算邻域点的最大高差,由于导线上的点高差理论上为0,计算邻域点高程极差Δh,筛除不符合的点,邻域点选择方法如图5(c)所示.
为进一步将结点处点云滤除并验证导线点云的线性特征,使用式(5)并设置更严格的阈值进一步剔除干扰点云.
1.2.2 站场钢轨顶面点云提取
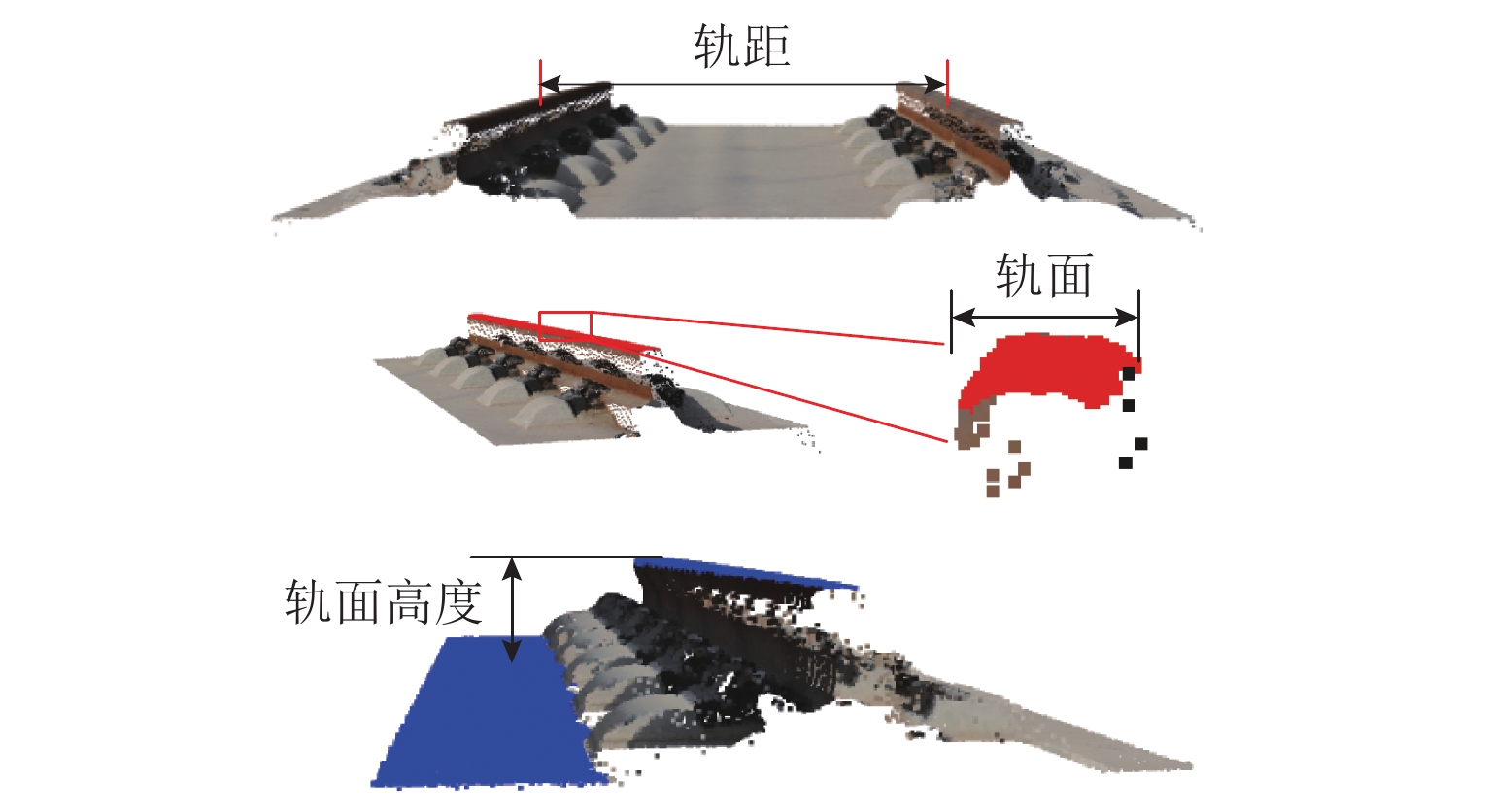
接触网导线特征的准确计算需要以钢轨顶面为基准. 钢轨具有明确、一致的建设规范要求的特征属性,P60钢轨点云模型如图6所示,用于钢轨顶面点云提取的知识约束条件. 约束条件包括:钢轨高于道床的距离、P60钢轨高度、站台钢轨区域点云密度在竖直维度上有陡然变化、钢轨轨距、轨顶面设计宽度、站场钢轨为两条彼此平行的直线. 这些约束条件为准确提取钢轨顶面点云提供了明确的几何与物理基础.
基于上述特征,提出一种主动采样的钢轨顶面点云鲁棒提取算法. 首先,利用钢轨顶面的法向量垂直向上进行约束,选择符合度好的钢轨点云. 随后,通过式(6)得到点云高程密度分布直方图. 利用轨面高度特点,找到钢轨顶面所在的大致范围,并通过该高度处点云密度是否显著变化,来验证所选取钢轨顶面点云数据的准确性.
h(Δx)=c(x,x+Δx)Δx, (6) 式中:Δx为高程微小范围差值,c(x,x+Δx)为特定高程范围[x,x+Δx]内点云数据点的计数,h(Δx)为在高程 x 处的点云密度.
结合钢轨直线分布特点,利用改进的RANSAC直线提取算法提取钢轨顶面点云数据,该算法利用钢轨轨面宽度、轨距以及钢轨间的高差约束规则来设置参数. 具体而言,RANSAC算法模型为:P(t)=P0+tV. 其中:P(t)为直线上任意一点;V 是沿钢轨方向的单位向量;t是一个实数,表示从P0点出发沿着钢轨方向向量V正向前进的位置.
1.2.3 站场接触网导线特征提取
站场接触网检测最重要的是提取导线高度(导高)和拉出值. 导线高度是指导线到钢轨顶面的垂直距离,拉出值是接触网导线对钢轨中心位置的水平偏移. 通过精确提取的导线与钢轨点云即可分别计算导高和拉出值. 首先,从接触网导线的点云数据中任意选取一个定位点;然后,基于最近邻搜索算法从离该定位点最近的2组钢轨顶面的点云数据中各选出J个最近点,并分别求取各组钢轨顶面的点云数据的三维坐标平均值ˉP1和ˉP2,再计算2个三维坐标平均值的高程平均值;最后,利用高程之差计算接触网导线的导高值.
接触网导线中某一个定位点的拉出值是接触网导线的定位点在钢轨顶面的投影点到轨道中心线段ˉP1ˉP2中点的距离. 由点到线段的投影公式,可得定位点到轨道中心线段的投影点坐标P′(xp,yp,zp),对应的钢轨匹配点对中点坐标M(xm,ym,zm). 由此,可计算出拉出值为
a=||P′M||=||P′−M||. (7) 2. 案例实验与分析
2.1 实验平台
为全面评估并验证本文所提算法的有效性和精度,以Visual Studio 2019为程序开发平台,使用PCL1.12.1进行辅助算法设计,实现基于点云数据的铁路站场接触网导线特征智能提取实验分析. 本文实验环境软硬件配置如表1所示.
表 1 软硬件配置Table 1. Hardware and software configuration环境配置 详细信息 硬件 CPU 12th Gen Intel Core i7-12700H 内存 16 GB 显卡 NVIDIA GeForce RTX 3060 软件 系统 Windows11 软件 PCL1.12.1、VS2019 2.2 数据来源
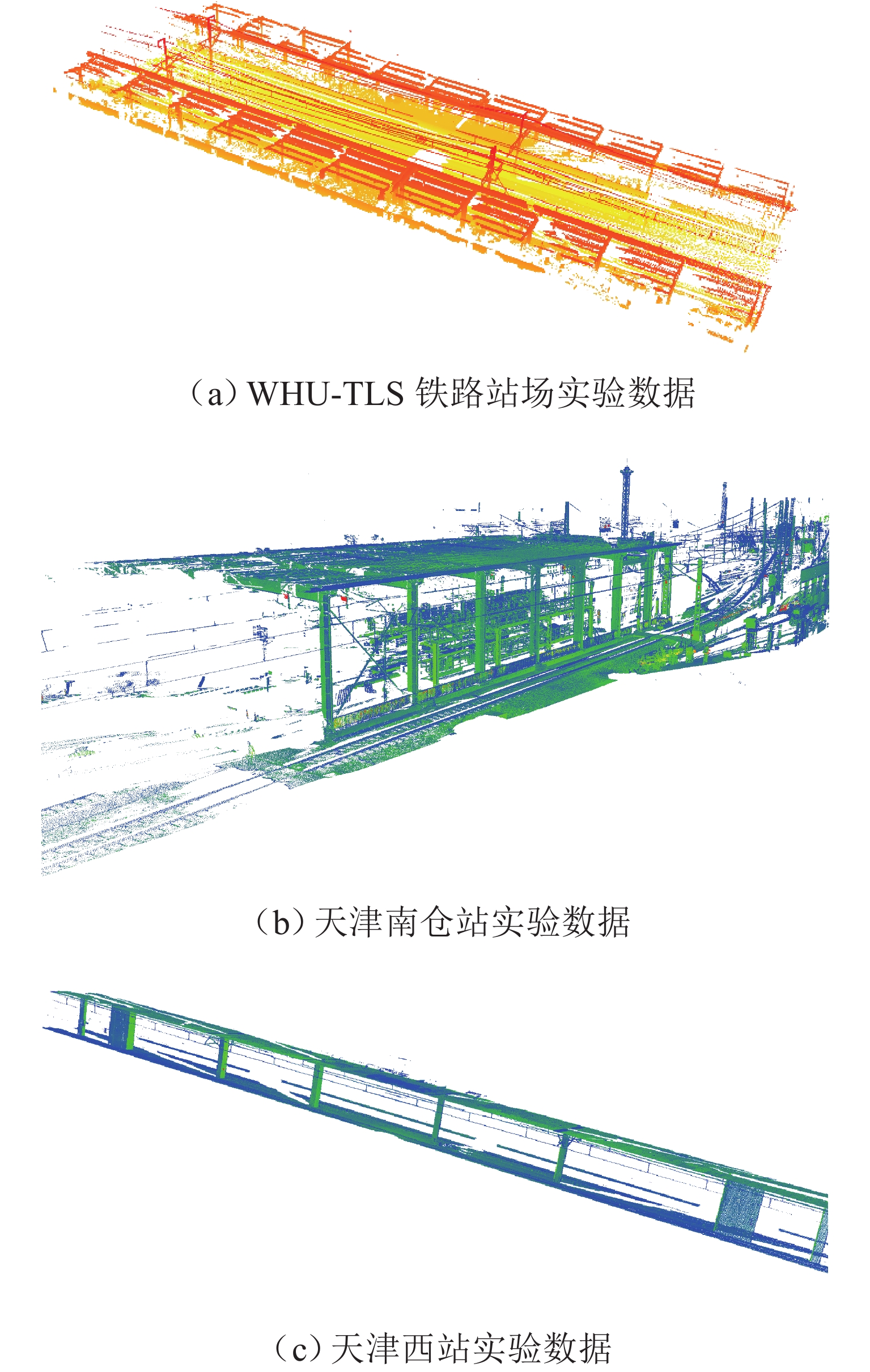
本文采用3组不同铁路站场接触网点云数据验证所提方法. 实验数据1来源于WHU-TLS铁路站场[28],使用的扫描仪是RIEGL VZ-400i,其最大精度可达5 mm,重复精度为3 mm. 以数据集中的第3个站点为例,预处理时去除与架设点较远且点集稀疏的区域,轨道方向上保留了长度不少于100 m的数据,单个架设点具有
6741169 个点,但其中存在部分数据缺失的情况,同时,点云密度也表现出分布不均匀等问题. 实验数据2为实测的天津南仓站场数据,用于辅助算法验证. 实验数据3是天津西站实测数据. 实验数据2与实验数据3均有大量的人工检测数据,用于校准实验结果. 所用数据在站场接触网运营维护案例中具有普遍代表性,能满足本文方法有效性和可行性验证的需求.2.3 实验结果
2.3.1 站场接触网激光点云预处理
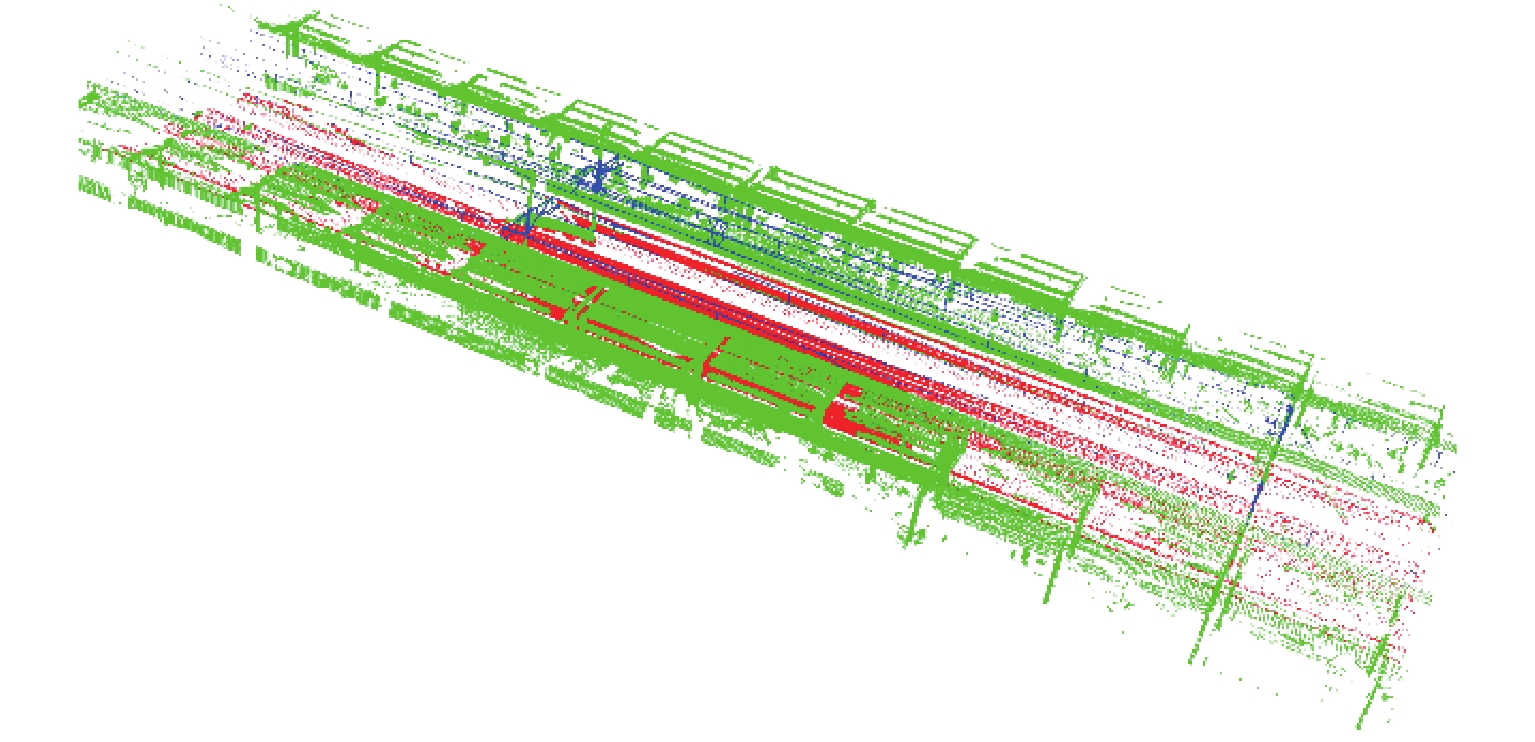
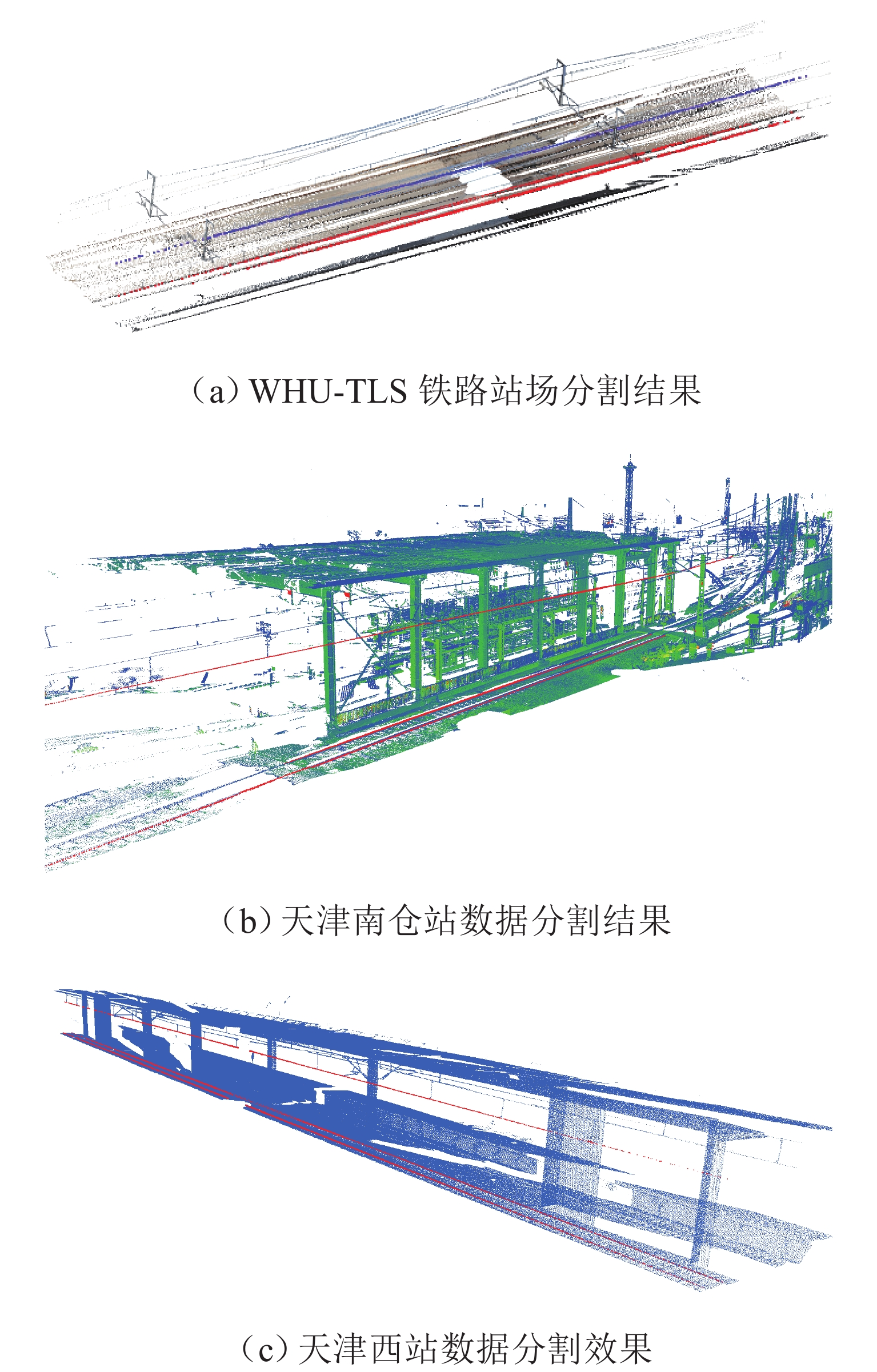
点云分类是预处理的首要步骤. 图7为3个站场场景实验段的原始点云,长度均超过100 m. 以WHU-TLS铁路站场实验数据为例,对该场景对象进行划分,结果如图8所示. 图中,红色点云为站场轨道部分(主要包括钢轨、轨枕、轨道板、砂浆层、底座等);蓝色部分是接触网主体结构,主要包括接触网导线、承力索、吊线、接触悬挂和部分支柱等;绿色部分是站台等背景点云. 然后,针对这3类建筑要素点云分别进行滤波处理. 对比滤波前、后(图7(a)和图8)的点云场景,滤波前的数据点为
5967092 个,滤波后的数据点为3863384 个.2.3.2 站场接触网导线与钢轨顶面提取
利用高程阈值和基于角度约束的环形邻域导线空间特征进行接触网导线粗提取,在处理吊弦与导线连接区域时,会面临如图9所示的分割难题(图9的放大视图中,3条沿竖直方向延伸的干扰点云与导线在空间分布和高程特征上高度相似,单纯利用阈值分割无法有效剔除). 为进一步优化提取效果,采用下半球半径邻域高差极差进行精细提取,经过验证,该方法能有效将接触网导线干扰点云去除,当邻域半径取0.04 m,Δh取0.02 m时,效果最佳. 针对难以滤除的结点,采用更加严格的λL阈值进一步滤除,得到图10所示结果,蓝色点云为提取的接触网导线点云.
利用高程和改进的RANSAC直线分割方法,分割点云顶面点云. 经过验证,当距离阈值设置为0.01 m时,可以很好地提取钢轨顶面中心直线,结果如图10所示,图中,红色点云为分割的钢轨顶面点云.
2.3.3 站场接触网导线分割算法对比
为评估本文方法在提取铁路站场接触网导线精度,将其与经典的RANSAC、3D Hough直线提取算法进行对比,从处理范围、耗时、鲁棒性和精度4个维度进行分析,如表2所示.
表 2 接触网导线点云分割算法对比Table 2. Accuracy of contact wire point cloud extraction方法 处理范围 耗时 鲁棒性 精确度 本文方法 长距离 最少 一般 最好 RANSAC 短距离 较多 较强 一般 3D Hough 短距离 最多 较强 最差 首先,考虑到接触网导线“Z”字形分布特点,在处理长距离接触网导线时,本文方法相比于传统直线提取算法耗时最少、精度最高. 但是在多个支柱之间时,由于接触网导线“Z”字形分布的特点,RANSAC和Hough算法无法一次性提取长距离接触网导线;接触网区域有吊弦等线状干扰点云,对Hough变换干扰较大. 其次,在处理相邻支柱的接触网导线时,RANSAC算法对离群点的鲁棒性表现优异. 最后,在精度方面,相邻支柱之间,本文方法和RANSAC算法精度较高,分割正确率可以达到95%以上.
尽管RANSAC、3D Hough直线提取算法在某些情况下表现出色,但其在处理复杂接触网场景时仍存在局限,本文方法则在这方面展现了较好的适应性和准确性.
2.3.4 站场接触网导线几何特征
随机选择10组数据,使用本文方法输出计算值,并与接触网线路智能动态参数检测装置(HR-CDS-Ⅱ型) 人工实测值进行对比,如表3所示. 表中,1~6组数据为天津西站数据,导高设计值为
5300 mm,7~10组为天津南仓站数据,导高设计值为6450 mm.表 3 接触网导线特征提取结果Table 3. Exaction results of contact wire featuresmm 组号 导高 实测
导高导高
差值拉出值 实测拉出值 拉出值差值 1 5306 5311 −5 193 199 −6 2 5300 5297 3 197 205 −8 3 5304 5310 −6 214 212 2 4 5304 5305 −1 220 218 2 5 5299 5294 5 223 223 0 6 5303 5297 6 230 231 −1 7 6449 6447 2 200 202 −2 8 6447 6450 −3 211 218 −7 9 6458 6464 −6 224 225 −1 10 6450 6456 −6 238 243 −5 根据《高速铁路接触网运行维修规则》可知[27],标准状态为标准值±30 mm. 此外,接触网导线拉出值处于标准状态时为设计值±30 mm,警戒值为400 mm,限界值为450 mm,实验段导高和拉出值均符合预设的标准限定值. 若一个定位点的站场接触网导线的几何特征大于警戒值时,则对接触网导线上该定位点的几何状态进行预警,从而为铁路管理人员提供决策服务.
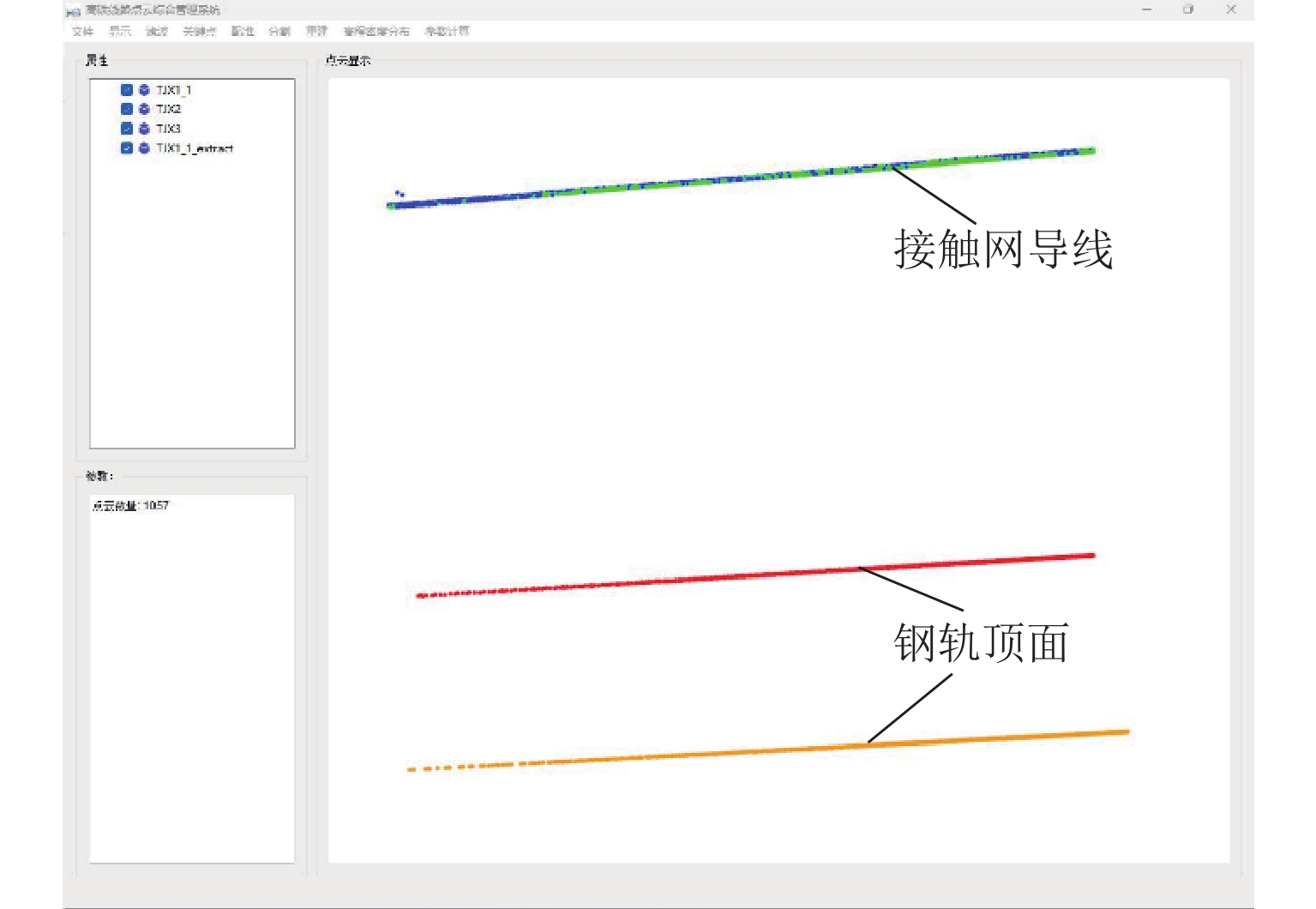
根据本文算法,最终构建原型系统如图11所示. 根据导高误差不同范围,赋予接触网导线不同的颜色显示,可以让管理者更直观地观察异常点位,从而保障站场接触网系统正常运行.
本文以2.2节所述3个铁路站场为案例进行实验. 在实验段100 m站场范围内,综合考虑到人工测量值的固有误差和接触网导线与设计值的自然偏差,本文方法提取的导线高度结果平均精度达到±5 mm,满足±10 mm误差范围的实际应用要求. 通过更精确的三维扫描方案能够进一步提升特征提取精度.
3. 结 论
1) 设计了一种站场关键要素点云的分割与融合滤波方法,在有效去除噪声的基础上,降低了点云数据量,提高了处理效率.
2) 利用铁路站场结构先验知识,设计了基于知识引导的点云精细分割策略,提出了一种利用角度约束的环形邻域与下半球半径邻域搜索策略相结合的方法,精确提取站场接触网导线点云数据.
3) 基于最近邻算法,将钢轨顶面点云与接触网导线点云一一匹配,准确提取接触网导线几何特征,平均精度达到±5 mm,表明本文方法能够实现导高、拉出值等导线几何特征的准确提取.
该方法可靠度高,易于操作并节省人力和时间成本,通过多个铁路站场接触网导线特征提取实验,证明了本文方法具有普适性与适用性,极具推广潜力. 后续研究中,将进行接触网场景点云多测站高精度配准研究,以更好地适应大范围铁路站场接触网场景. 值得一提的是,通过进一步改进点云邻域,例如圆锥邻域,有望在接触网导线分割获得更好的结果,也可适用于部分站外曲线轨道场景.
致谢:中国铁路北京局集团有限公司科技研究开发计划(2022AGD01)、西成铁路客运专线陕西有限责任公司科技研究开发计划项目(西康高铁合(2021)24号)的资助.
-
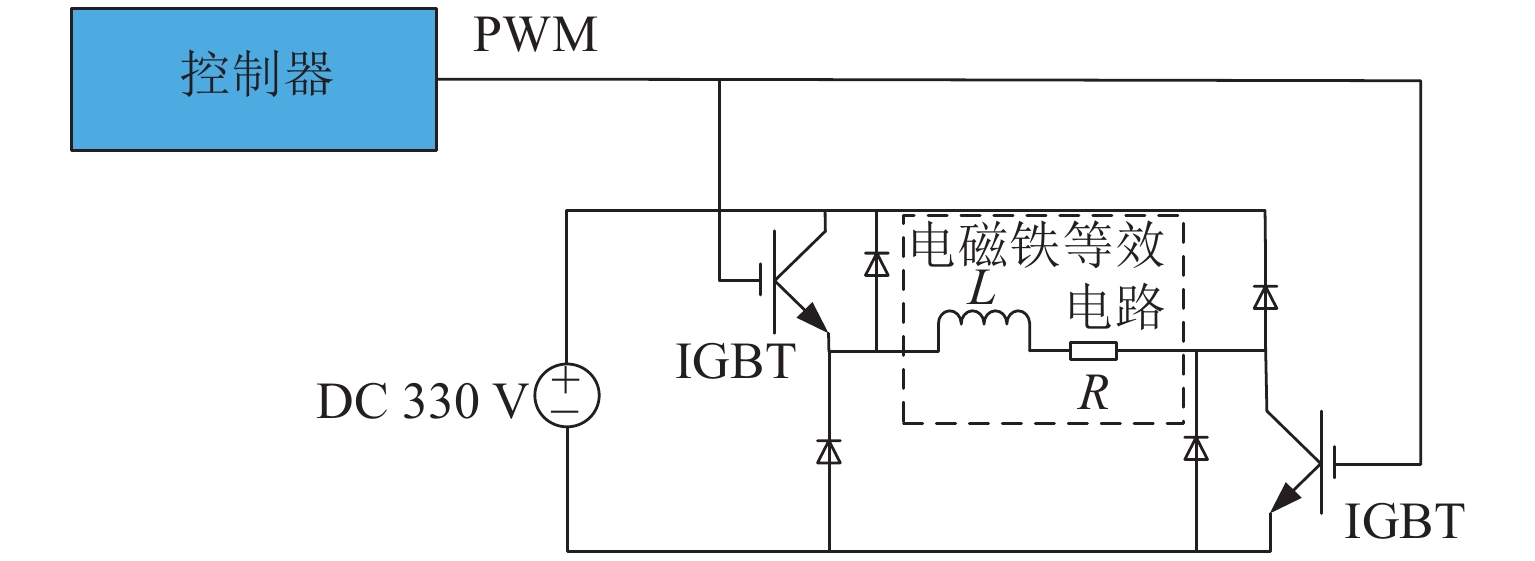
表 1 悬浮架参数
Table 1. Parameters of levitation frame
参 数 数 值 等效质量/kg 510 电磁铁等效内阻/Ω 0.98 电磁铁等效电感/mH 36 电磁铁匝数 N 640 正对横截面积/m2 0.078 4 真空磁导率/(H·m−1) 4π × 10−7 表 2 仿真中不同参考信号算法误差的对比
Table 2. Comparison of reference signal errors of different algorithms in simulation
参考信号
控制器正常悬浮 方波信号 正弦信号 EITAE EIAE ERMSE EITAE EI AE ERMSE EITAE EIAE ERMSE PID 38.67 3.82 0.24 38.68 3.82 0.24 38.68 3.82 0.24 LQR 51.20 5.10 0.28 51.44 5.12 0.28 51.43 5.11 0.28 MRAC 16.72 1.69 0.11 34.34 3.3 0.22 164.78 16.44 0.92 SMRAC 19.51 1.97 0.12 43.23 4.28 0.27 128.23 13.37 0.76 表 3 仿真中不同算法上升时间和调节时间对比
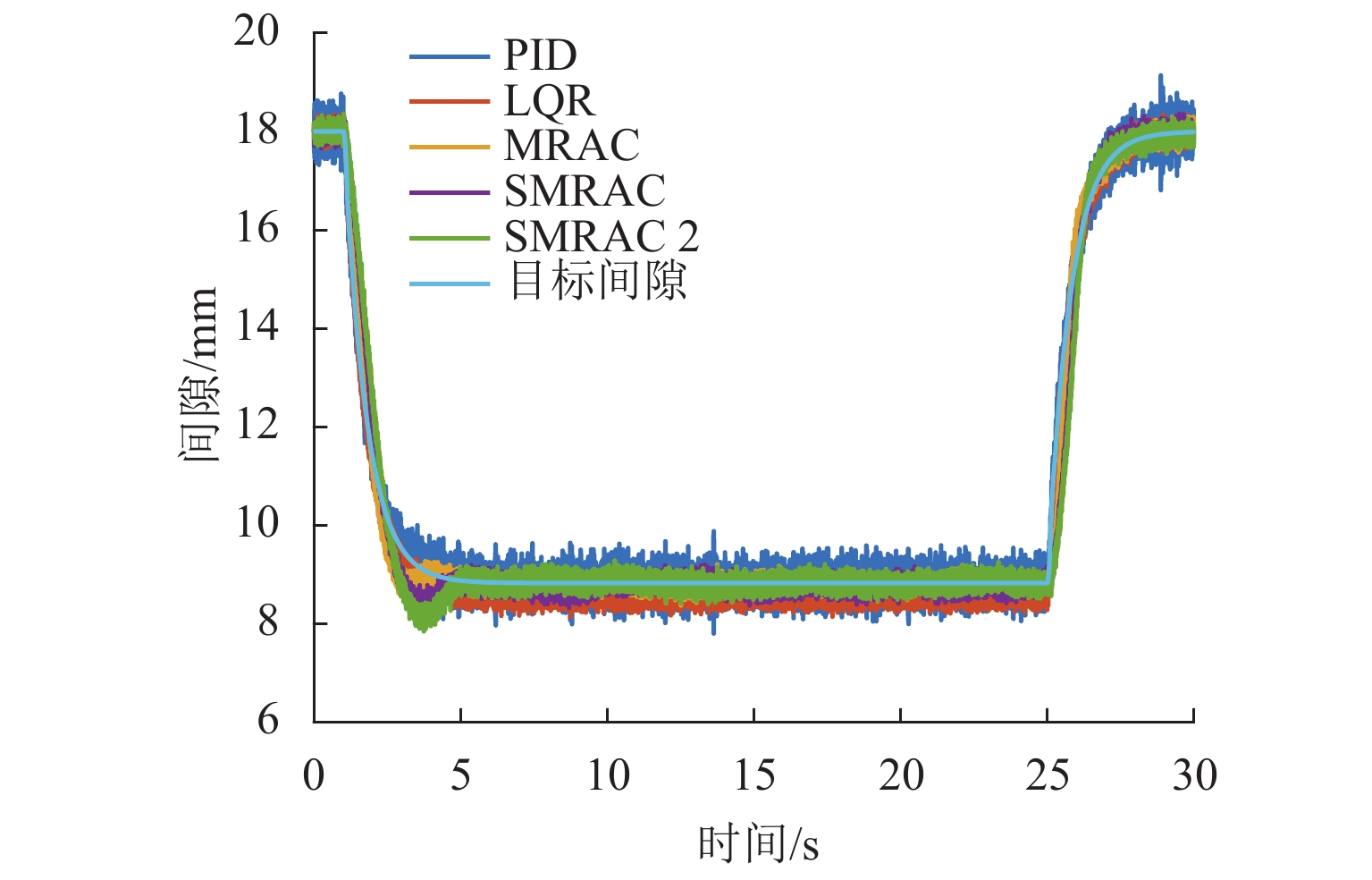
Table 3. Comparison of rising time and adjustment time of different algorithms in simulation
s 控制算法 上升时间 调节时间 PID 1.57 2.03 LQR 1.40 1.71 MRAC 1.57 2.03 SMRAC 1.02 1.41 表 4 悬浮架质量变为 3 倍后不同算法的误差对比
Table 4. Comparison of different algorithms after mass increase by three times
控制算法 PID LQR MRAC SMRAC EITAE 35.10 51.07 26.55 57.22 EIAE 3.54 5.09 2.70 7.23 ERMSE 0.22 0.28 0.17 0.45 表 5 质量增加3倍后不同算法的上升时间和调节时间
Table 5. Rising time and adjustment time of different algorithms after mass increase by three times
s 控制算法 上升时间 调节时间 PID 1.84 2.02 LQR 1.57 2.02 MRAC 1.37 1.74 SMRAC 1.57 1.78 表 6 实测中不同算法的性能对比
Table 6. Comparison of performance indexes in experiment
控制算法 EITAE EIAE ERMSE PID 57.57 3.38 0.16 LQR 83.72 4.82 0.19 MRAC 79.78 4.13 0.22 SMRAC 77.15 4.15 0.21 表 7 实测中不同算法的上升时间和调节时间
Table 7. Rising time and adjustment time of different algorithms in experiment
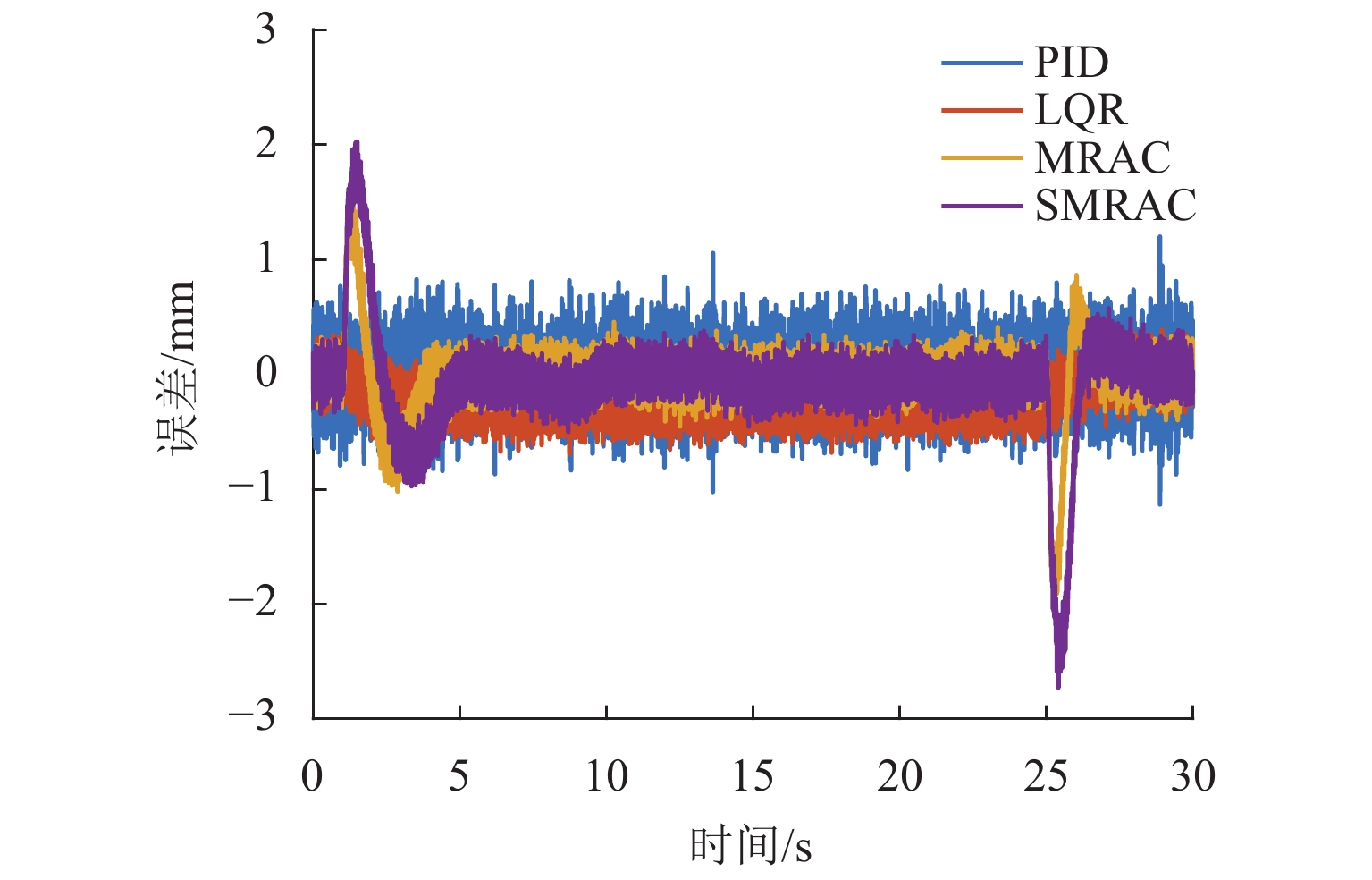
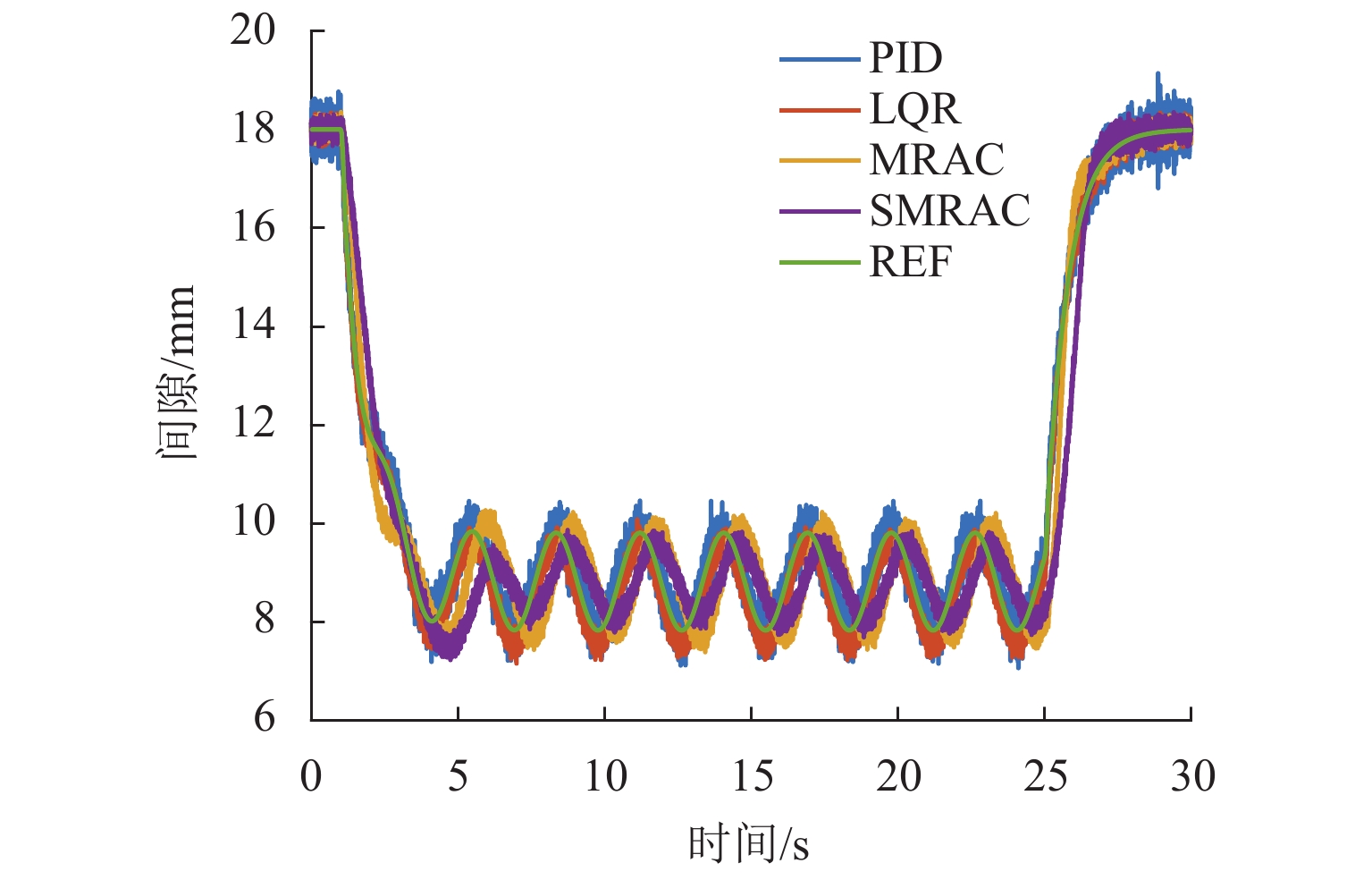
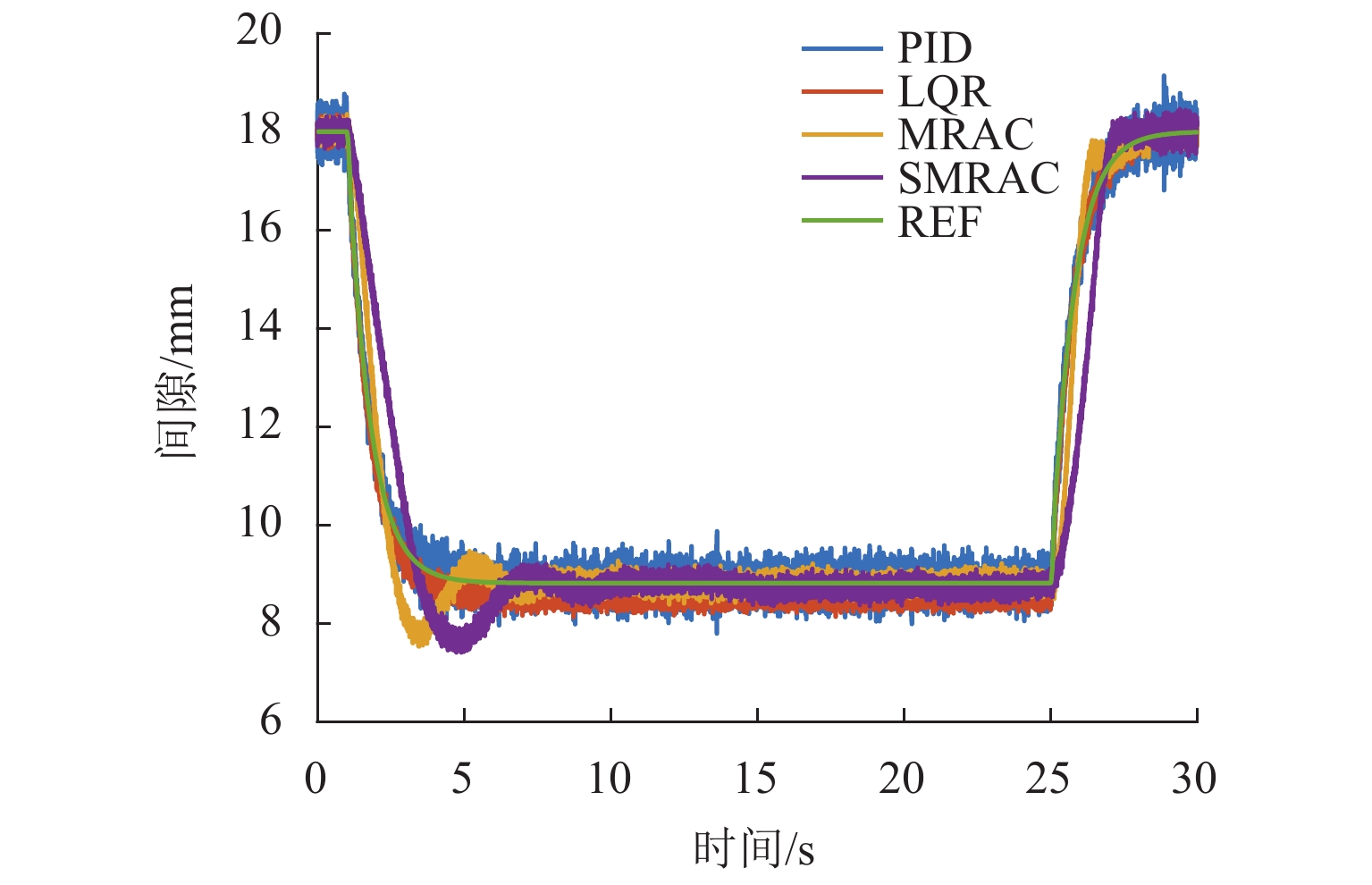
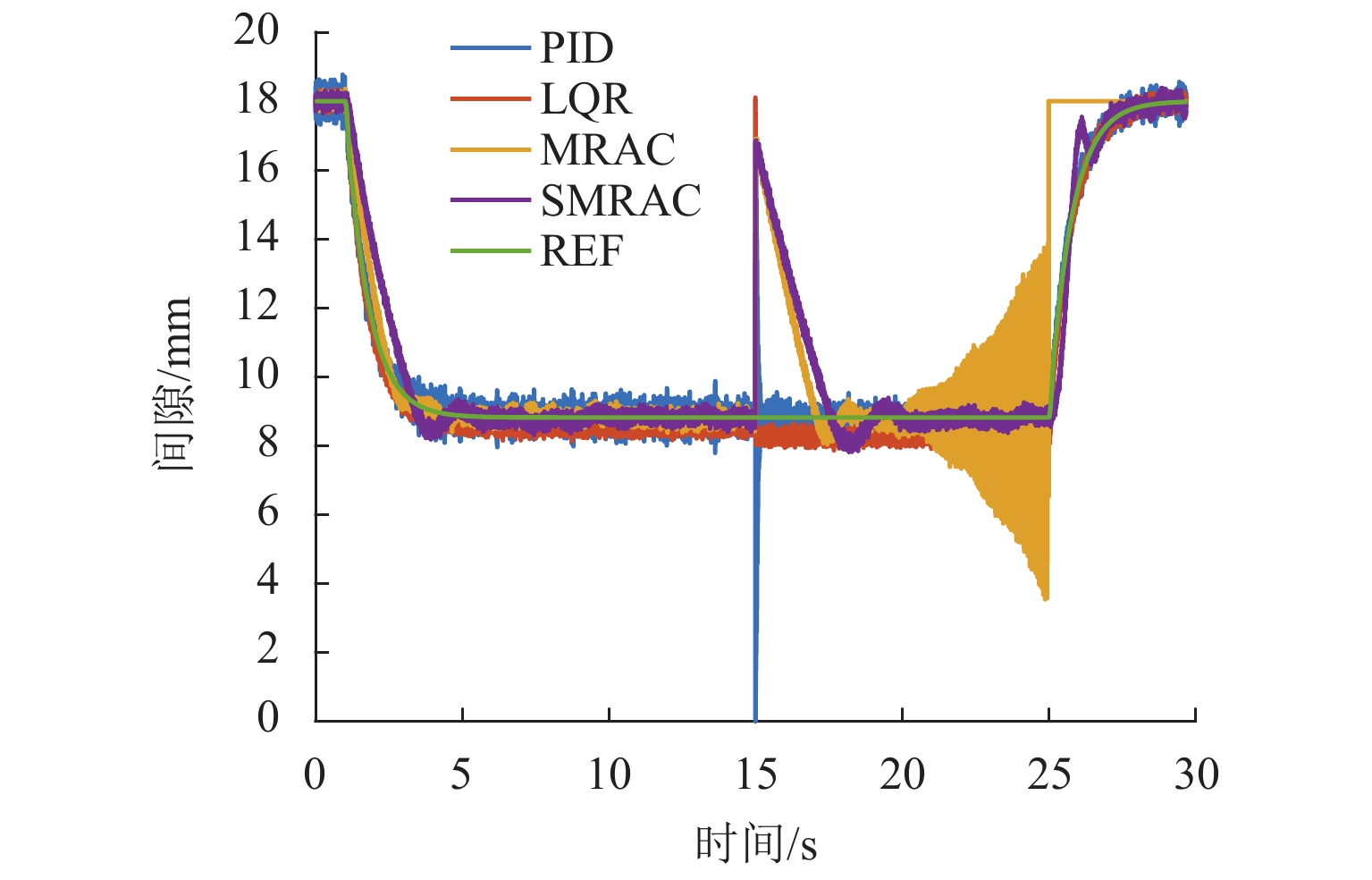
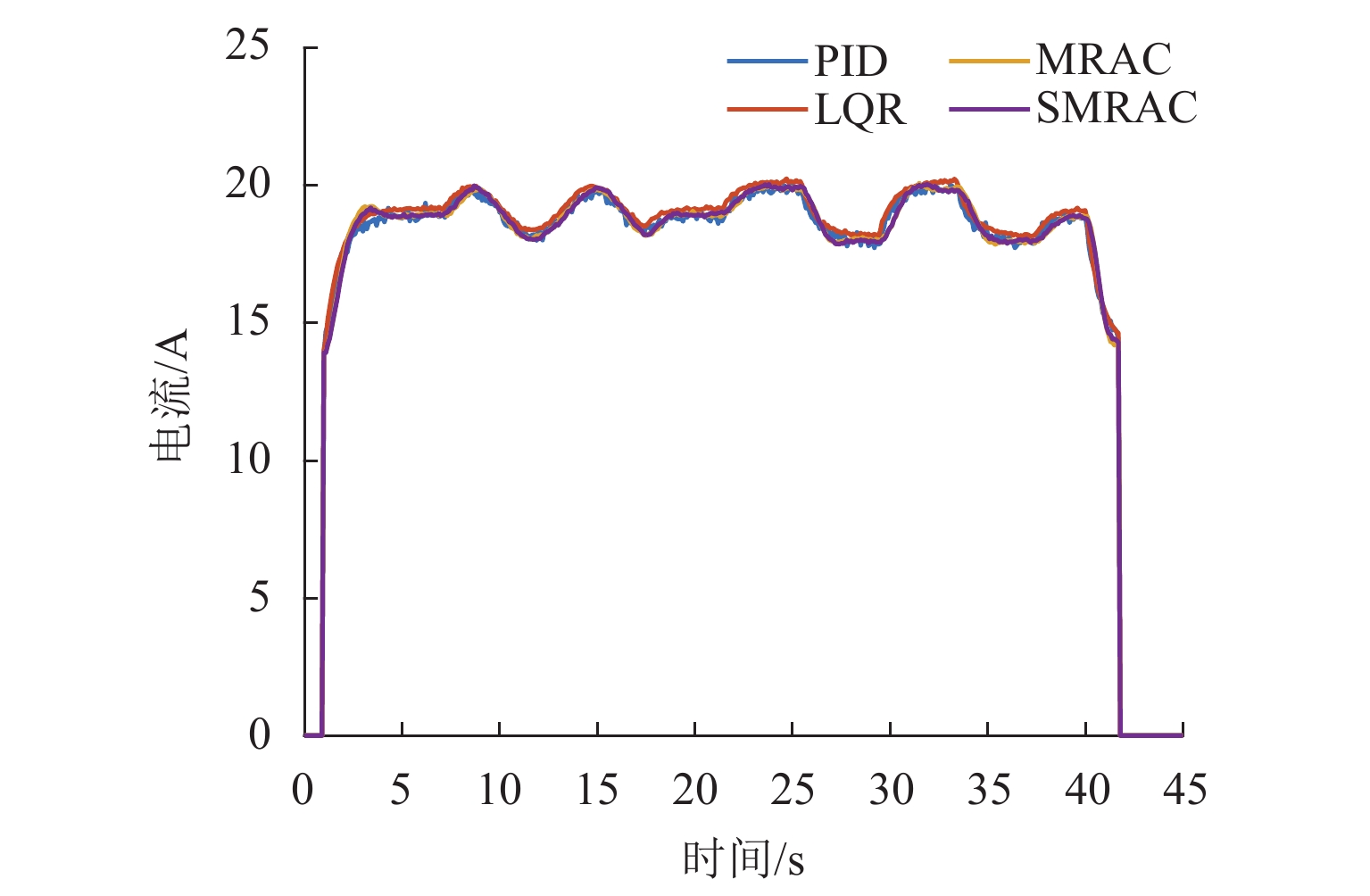
s 控制算法 上升时间 调节时间 PID 2.20 2.80 LQR 1.41 1.70 MRAC 1.47 2.06 SMRAC 1.21 2.04 -
[1] 马卫华,罗世辉,张敏,等. 中低速磁浮车辆研究综述[J]. 交通运输工程学报,2021,21(1): 199-216.MA Weihua, LUO Shihui, ZHANG Min, et al. Research review on medium and low speed maglev vehicle[J]. Journal of Traffic and Transportation Engineering, 2021, 21(1): 199-216. [2] SCHMID P, EBERHARD P. Offset-free nonlinear model predictive control by the example of maglev vehicles[J]. IFAC-PapersOnLine, 2021, 54(6): 83-90. doi: 10.1016/j.ifacol.2021.08.528 [3] SHI Y, MA W H, LI M A, et al. Research on dynamics of a new high-speed maglev vehicle[J]. Vehicle System Dynamics, 2022, 60(3): 721-742. doi: 10.1080/00423114.2020.1838568 [4] NI F, MU S Y, KANG J S, et al. Robust controller design for maglev suspension systems based on improved suspension force model[J]. IEEE Transactions on Transportation Electrification, 2021, 7(3): 1765-1779. doi: 10.1109/TTE.2021.3058137 [5] WAHYUDIE A, SUSILO T B, ALI NANDAR C S, et al. Simple robust PID tuning for magnetic levitation systems using model-free control and H-infinity control strategies[J]. International Journal of Control, Automation and Systems, 2021, 19(12): 3956-3966. doi: 10.1007/s12555-020-0253-8 [6] LI B W, LI X L, LONG Z Q. Design of model reference adaptive controller for active guidance system of high speed maglev train[C]//2020 Chinese Control and Decision Conference (CCDC). Hefei: IEEE, 2020: 945-950. [7] 张锟,崔鹏,李杰. 磁悬浮系统的加速度计反馈控制算法[J]. 控制理论与应用,2009,26(9): 988-992.ZHANG Kun, CUI Peng, LI Jie. Accelerometer feedback control algorithm for maglev system[J]. Control Theory & Applications, 2009, 26(9): 988-992. [8] WEI W, XUE W C, LI D H. On disturbance rejection in magnetic levitation[J]. Control Engineering Practice, 2019, 82: 24-35. doi: 10.1016/j.conengprac.2018.09.018 [9] LI B W, LI X L, WANG Z Q, et al. Design of ADRC for guidance system of high speed maglev train[C]//2020 Chinese Automation Congress (CAC). Shanghai: IEEE, 2021: 1471-1476. [10] 靖永志,冯伟,王森,等. 基于自适应非奇异终端滑模的悬浮控制策略[J]. 西南交通大学学报,2022,57(3): 566-573.JING Yongzhi, FENG Wei, WANG Sen, et al. Levitation control strategy based on adaptive non-singular terminal sliding mode[J]. Journal of Southwest Jiaotong University, 2022, 57(3): 566-573. [11] SUN Y G, XU J Q, ZHANG W Y, et al. Neural network-based adaptive control for EMS type maglev vehicle systems with time-varying mass[C]//International Conference on Neural Computing for Advanced Applications. Singapore: Springer, 2020: 381-394. [12] 陈萍,史天成,于明月,等. 中低速磁浮列车滑模自抗扰悬浮控制算法[J]. 铁道科学与工程学报,2023,20(2): 682-693.CHEN Ping, SHI Tiancheng, YU Mingyue, et al. Sliding mode active disturbance rejection levitation control algorithm of the medium-and low-speed maglev vehicles[J]. Journal of Railway Science and Engineering, 2023, 20(2): 682-693. [13] LI S Q, ZHANG K L, LIU G Q, et al. EMS maglev vehicles model reference adaptive control[C]//2015 34th Chinese Control Conference (CCC). Hangzhou: IEEE, 2015: 2989-2993. [14] WANG H M, GE X L, LIU Y C. Second-order sliding-mode MRAS observer-based sensorless vector control of linear induction motor drives for medium-low speed maglev applications[J]. IEEE Transactions on Industrial Electronics, 2018, 65(12): 9938-9952. doi: 10.1109/TIE.2018.2818664 [15] 曾国保. 中低速磁浮交通的适应性及工程化发展方向[J]. 铁道工程学报,2016,33(10): 111-115. doi: 10.3969/j.issn.1006-2106.2016.10.023ZENG Guobao. The adaptability and the improvement in engineering of the lower-medium speed maglev transit system[J]. Journal of Railway Engineering Society, 2016, 33(10): 111-115. doi: 10.3969/j.issn.1006-2106.2016.10.023 [16] 曹广忠. 磁悬浮系统控制算法及实现[M]. 北京: 清华大学出版社, 2013. [17] ZHANG M, LUO S H, GAO C, et al. Research on the mechanism of a newly developed levitation frame with mid-set air spring[J]. Vehicle System Dynamics, 2018, 56(12): 1797-1816. doi: 10.1080/00423114.2018.1435892 [18] 刘清辉, 单磊, 马卫华, 等. 考虑剩磁作用的中低速磁浮电磁力分析[J/OL]. 西南交通大学学报, [2022-9-25]. https://kns.cnki.net/kcms2/article/abstract?v=3uoqIhG8C45S0n9fL2suRadTyEVl2pW9UrhTDCdPD65IXe8xbsrGOD399GtDO21yEnpsZPB2dzXbpP36DTmjFAOzm3-A2U4V&uniplatform=NZKPT. [19] XU J Q, SUN Y G, GAO D G, et al. Dynamic modeling and adaptive sliding mode control for a maglev train system based on a magnetic flux observer[J]. IEEE Access, 2018, 6: 31571-31579. doi: 10.1109/ACCESS.2018.2836348 [20] JIBRIL M. Comparisons of fuzzy MRAS and PID controllers for EMS maglev train[J]. Information and Knowledge Management, 2020, 10(4): 34-40. [21] 孙凤, 邢大壮, 周冉, 等. 考虑能耗的电磁主动悬架 LQR 控制[J/OL]. 西南交通大学学报. [2023-4-3]. https://kns.cnki.net/ kcms/detail/51.1277.U.20230329.1507.006.html. -






 下载:
下载:











 下载:
下载: