Analysis of Bending Natural Vibration Characteristics of Box Girder Based on Additional Deflection for Shear Lag
-
摘要:
为揭示弯曲空间效应对自振频率削弱的影响规律,选取剪力滞效应引起的附加挠度为广义位移,将箱梁翘曲附加变形纳入体系总动能中,运用Hamilton原理,建立考虑剪切、剪力滞及双重效应影响的箱梁弯曲自振频率变分解析解,引入空间效应对自振频率削弱影响的差值比参数,详细分析截面尺寸及边中跨径比对差值比参数的影响. 算例分析表明:考虑剪切和双重效应影响的箱梁自振频率解析解与有限元数值解吻合较好;频率阶数越大,各效应对自振频率的削弱程度越大,其中,双重效应影响最为显著,对一阶频率,双重效应对简支和连续箱梁自振频率分别削弱了4.72%和4.80%;跨宽比、宽高比和边中跨径比越大,自振频率差值比越小;板宽比越大,剪力滞、双重效应自振频率差值比越小,剪切自振频率差值比越大;同一跨宽比时,剪力滞和剪切效应对自振频率的削弱程度相当;不同宽高比下,剪力滞效应对自振频率的削弱程度近乎相同,剪切效应影响较为显著;低阶自振频率计算时可按不带悬臂板的箱梁进行计算.
Abstract:In order to reveal the influence of the bending space effect on natural vibration frequency weakening, the additional deflection caused by the shear lag effect was selected as the generalized displacement, and the warping additional deformation of box girder was incorporated into the total kinetic energy of the system. The bending variational solution of the natural vibration frequency of box girder considering the influence of shear deformation, shear lag, and their double effects was established by using the Hamilton principle. The difference ratio parameter of spatial effect on natural vibration frequency weakening was introduced, and the influence of the section size and the side-to-middle span on the difference ratio parameter was analyzed in detail. The example analysis shows that the analytical solution of the natural vibration frequency of the box girder considering shear derformation and double effects is in good agreement with the finite element numerical solution. A larger frequency order indicates a greater weakening degree of each effect to the natural vibration frequency. The double effect is the most significant, which reduces the natural vibration frequency of simply supported and continuous box girders by 4.72% and 4.80% respectively for the first-order frequency. The larger span width ratio, width-to-height ratio, and side-to-middle span ratio indicate a smaller difference ratio of natural vibration frequency. A larger plate width ratio is accompanied by a smaller difference ratio of natural vibration frequency considering shear lag and double effect and a larger difference ratio of natural vibration frequency considering shear derformation . At the same span width ratio, shear lag and shear derformation effects weaken the natural vibration frequency to the same extent. At the different width-to-height ratio ratios, the shear lag effect weakens the natural vibration frequency to almost the same extent, and the shear derformation effect has a more significant impact. Low-order natural vibration frequency can be calculated using a box girder without a cantilever plate.
-
箱梁因其良好的受力特性被广泛应用于现代桥梁工程中. 结构自振频率是衡量动力特性的重要指标之一,其将为箱梁桥抗风、抗震设计及结构健康监测等提供重要的理论基础[1]. 箱梁桥自振特性分析方法有能量变分法[2-3]、矩阵分析法[4-5]、有限元法[6]和试验法等[7-8],其中,能量变分法是研究箱梁桥自振频率最常用的一种解析方法. 箱梁自振特性包括弯曲和扭转2种基本变形[9],而弯曲自振特性是箱梁桥健康监测及全寿命周期预测的重要表征数据之一. 近年来,国内外学者在箱梁弯曲自振特性方面,已取得了一系列丰富的研究成果: Jiang等[10]运用Hamilton原理建立考虑剪切变形、剪力滞和转动惯量等影响的箱梁弯曲固有频率解析法;李丽园等[11]建立了统筹考虑全截面剪切变形影响的连续箱梁自振频率变分解;周茂定等[12]基于力法原理推导了箱梁各板面内的剪切系数表达式,并分析了各板剪切变形对弯曲自振频率的影响;Zhang等[13]推导了考虑剪力滞和手风琴效应影响的波纹钢腹板组合箱梁弯曲自振特性变分解,并揭示了2种效应对弯曲自振频率的影响规律;项贻强等[14]基于铁木辛柯梁理论和组合梁滑移理论,建立考虑体外预应力束影响的钢-混组合小箱梁动力特性解析解,并分析预加力对箱梁自振特性的影响;Shen等[15]基于高阶理论和Carrera闭合解,分析钢-混凝土组合梁的自由振动和应力状态;冀伟等[16]运用Galerkin法和Hamilton原理,分析剪切和剪力滞效应对新型波形钢腹板组合箱梁弯曲自振频率的影响. 此外,还有学者研究了温度对箱梁自振特性的影响[17]及系杆拱桥的自振特性等[18].
上述研究在建立考虑剪力滞影响的箱梁弯曲自振频率变分解时,常以最大剪切转角差为广义位移,且未计入翘曲附加变形对箱梁总动能的影响;其次,该广义位移物理意义不明确,且不便于直接获得剪力滞引起的箱梁桥弯曲动力响应. 本文提出一种基于剪力滞附加挠度的箱梁弯曲自振特性变分解析法,详细分析箱梁几何参数对频率差值比的影响,研究成果为箱梁桥动力特性参数的精确计算和合理设计提供理论依据.
1. 箱梁弯曲自振频率
1.1 考虑剪切剪力滞影响的弯曲自振频率
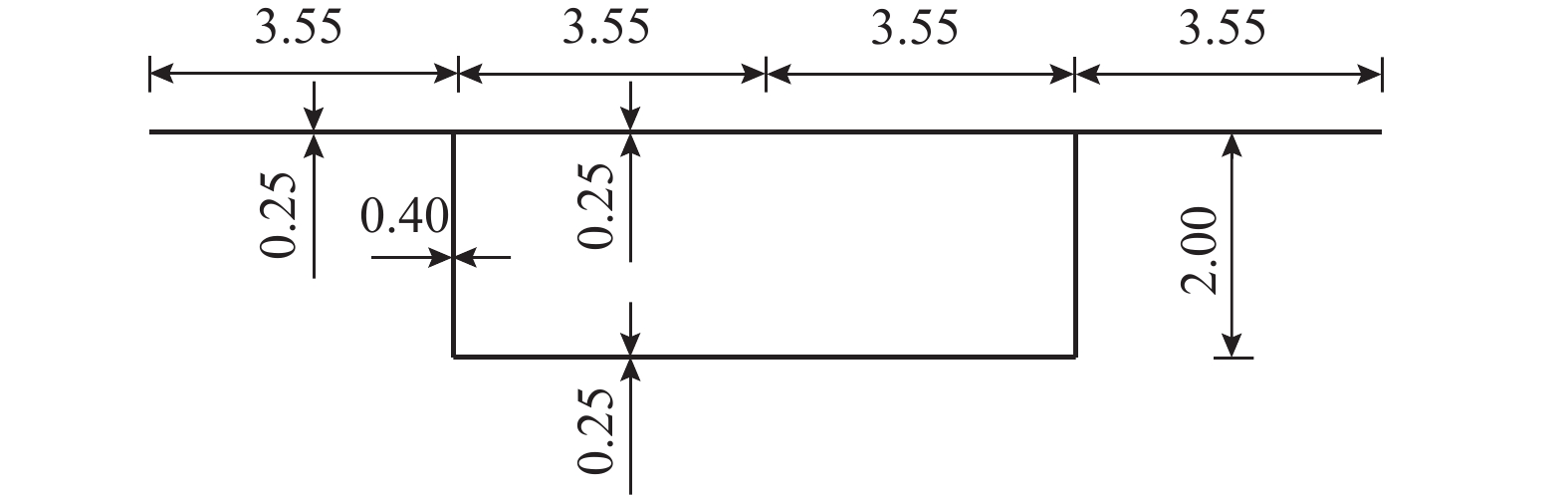
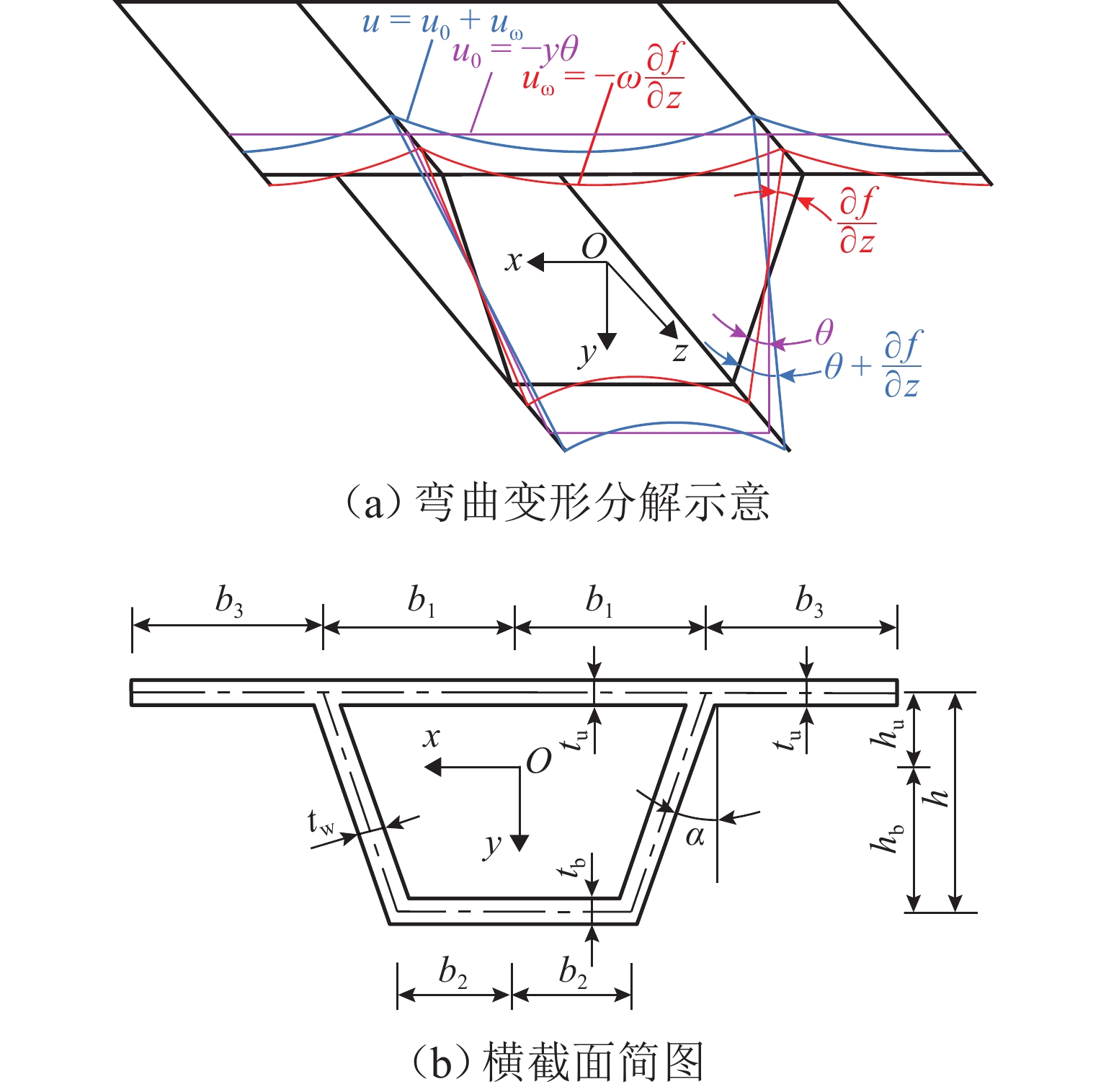
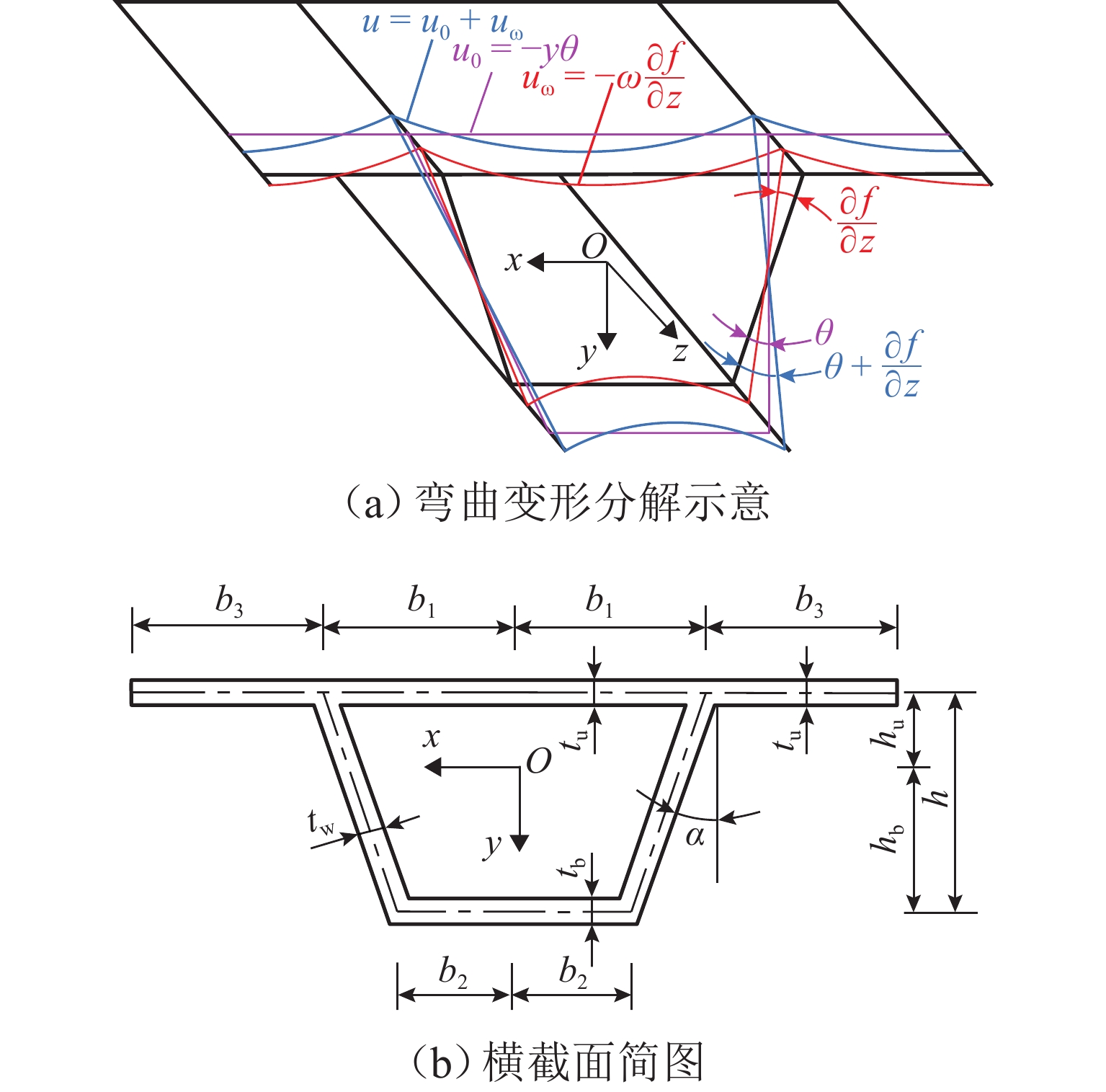
在直角坐标系O-xyz下,结合弹性叠加原理,将箱梁弯曲变形分解为考虑腹板剪切影响的初等梁变形和剪力滞翘曲变形,如图1(a)所示,横截面如图1(b)所示. 图中:u为箱梁纵向位移,u0和θ分别为考虑剪切变形的截面纵向位移和转角,uω为剪力滞翘曲纵向位移,f为附加挠度,l为箱梁跨度,2b1、2b2、b3分别为顶、底板宽和单侧悬臂板宽,hu和hb分别为顶、底板中面至中和轴的距离,h为顶、底板中面之间的距离,tu、tb和tw分别为顶、底板厚度和腹板厚度,α为腹板俯角,ω为翘曲位移分布函数.
以剪力滞附加挠度f (z)为广义位移,则考虑剪切剪力滞效应(以下简称双重效应)后箱梁截面上任一点的纵向位移为
u(x,y,z)=u0+uω=−yθ−ω∂f(z)∂z, (1) ω ={hucos(πx2b1)+η,顶板,−hbcos(πx2b2)b2b1hbhu+η,底板,hucos(π(b1+b3−x)2b3)b3b1ϕ+η,悬臂板,η,腹板, (2) 式中:η为翘曲正应力自平衡系数,通过$\int {\omega {\text{d}}A} {\text{ = }} 0$求得,A为横截面积;ϕ为悬臂板边界约束修正系数,可取1.4[19].
结合弹性理论及铁木辛柯梁理论[14],考虑双重效应的箱梁体系应变能为
Π=EIx2∫l0(∂θ∂z)2dz+EIω2∫l0(∂2f∂z2)2dz+EIyω∫l0∂θ∂z∂2f∂z2dz+GAω2∫l0(∂f∂z)2dz+GAw2∫l0(∂w∂z−θ)2dz, (3) 式中:前3项为弯翘正应变能,第4项为剪力滞翘曲剪切应变能,第5项为腹板剪切应变能;E为弹性模量;G为剪切模量;Ix、Iω分别为抗弯惯性矩和翘曲惯性矩,${I_{\text{ω}} } = \int {{\omega ^2}{\text{d}}A}$;Iyω为翘曲惯性积,${I_{{\rm{y}}{\text{ω}} }} = \int {y\omega {\text{d}}A}$;Aw为腹板横截面积;Aω为翘曲面积,${A_{{{\text{ω}}}} } = \int {{{\left( \dfrac{{{\partial}} {\omega} }{\partial {x}} \right)}^{2}}{\text{d}}A}$.
为准确分析剪力滞效应对箱梁动力特性的影响,将剪力滞附加变形纳入箱梁总动能T中,即
T=ˉm2∫l0((∂w∂t)2+(∂f∂t)2) dz, (4) 式中:$ \bar m $为每延米箱梁的自重,由材料质量密度ρ和横截面积A确定;w为竖向挠度.
运用Hamilton原理[10,14]对箱梁体系总能量进行一阶变分运算,并令${\text{δ}} \displaystyle\int_{{t_1}}^{{t_2}} {(T - \varPi )} {\text{d}}t = 0$得
δ∫t2t1(T−Π)dt=∫t2t1∫l0[ˉm(∂w∂tδ∂w∂t+∂f∂tδ∂f∂t)−EIx∂θ∂zδ∂θ∂z−EIω∂2f∂z2δ∂2f∂z2−GAω∂f∂zδ∂f∂z−EIyω(∂θ∂zδ∂2f∂z2+∂2f∂z2δ∂θ∂z)−GAw(∂w∂z−θ)δ(∂w∂z−θ)]dzdt=0, (5) 式中:t1、t2分别为起止、时间.
整理式(5)可得考虑双重效应影响的箱梁自由振动控制微分方程为
−ˉm∂2w∂t2+GAw(∂2w∂z2−∂θ∂z)=0, (6) −ˉm∂2f∂t2−EIω∂4f∂z4−EIyω∂3θ∂z3+GAω∂2f∂z2=0, (7) EIx∂2θ∂z2+EIyω∂3f∂z3+GAw(∂w∂z−θ)=0. (8) 式(5)变分所需的边界条件为
{−(EIx∂θ∂z+EIyω∂2f∂z2)δθ|l0=0,−(EIω∂2f∂z2+EIyω∂θ∂z)δ∂f∂z|l0=0,−(EIω∂3f∂z3+EIyω∂2θ∂z2−GAω∂f∂z)δf|l0=0,−GAw(∂w∂z−θ)δw|l0=0. (9) 式(9)中的第3个边界条件对δf变分,根据变分边界项物理意义,其对应翘曲广义剪力为
Qω=−EIω(∂3f∂z3+ζ∂2θ∂z2−k2∂f∂z), (10) 式中:k为瑞斯勒参数,$k = \sqrt {\dfrac{{G{A_\omega }}}{{E{I_\omega }}}} $;$\zeta = \dfrac{{{I_{{\rm{y}}{\text{ω}} }}}}{{{I_{\text{ω}} }}}$.
令w(z,t)=w(z)sin$(\hat\omega t $+ψ),θ(z,t)=θ(z)sin$(\hat\omega t $+ψ),f(z,t)=f(z)sin($\hat\omega t$+ψ),其中,$\hat\omega $和ψ分别为箱梁自振圆频率和初始相位角. 将其代入式(6) ~ (8)可得
ˉmˆω2w+GAw(∂2w∂z2−∂θ∂z)=0, (11) ˉmˆω2f−EIω∂4f∂z4−EIyω∂3θ∂z3+GAω∂2f∂z2=0, (12) EIx∂2θ∂z2+EIyω∂3f∂z3+GAw(∂w∂z−θ)=0. (13) 整理式(11) ~ (13),可得w的控制微分方程,即
∂8w∂z8+(J1ˉmˆω2−J2)∂6w∂z6−J3ˉmˆω2∂4w∂z4+(J4−J5ˉmˆω2)ˉmˆω2∂2w∂z2+J6ˉm2ˆω4w=0, (14) 式中:${J_1} = \dfrac{1}{{G{A_{\rm{w}}}}}$,${J_2} = \dfrac{{G{A_{\text{ω}} }{I_{\rm{x}}}}}{{E({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2)}}$,${J_3} = \dfrac{{{I_{\rm{x}}} + {I_{\text{ω}} }}}{{E({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2)}} +$$\dfrac{{{I_{\rm{x}}}{A_{\text{ω}} }}}{{E({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2){A_{\rm{w}}}}}$,${J_4} = \dfrac{{G{A_{\text{ω}} }}}{{{E^2}({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2)}}$, ${J_5} = \dfrac{{{I_{\rm{x}}}}}{{G{A_{\rm{w}}}E({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2)}}$,${J_6} = \dfrac{1}{{{E^2}({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2)}}$.
求解式(14),可得考虑剪切变形影响的箱梁挠度为
ws(z)=C1sin(λ1z)+C2cos(λ1z)+ C3sin(λ2z)+C4cos(λ2z)+C5sinh(λ3z)+ C6cosh(λ3z)+C7sinh(λ4z)+C8cosh(λ4z), (15) 式中:C1~C8为挠度待定系数,由边界条件确定;λ1~λ4为特征方程根.
将式(15)代入式(11)后,根据恒等式原理,可导出
θ(z)=B1(C1cos(λ1z)−C2sin(λ1z))+B2(C3cos(λ2z)−C4sin(λ2z))+B3(C5cosh(λ3z)+C6sinh(λ3z))+B4(C7cosh(λ4z)+C8sinh(λ4z)), (16) 式中:B1~B4为转角系数,即${B_i} = {\lambda _i}\left(1 - \dfrac{{\bar m{\hat\omega ^2}}}{{G{A_{\rm{w}}}\lambda _i^2}}\right)$,i=1,2,${B_j} = {\lambda _j}\left(1 + \dfrac{{\bar m{{\hat\omega} ^2}}}{{G{A_{\rm{w}}}\lambda _j^2}}\right)$,j=3,4.
整理式(12)、(13),并将ws、θ代入后,可得
f(z)=D1(C1sin(λ1z)+C2cos(λ1z))+D2(C3sin(λ2z)+C4cos(λ2z))+D3(C5sinh(λ3z)+⋅C6cosh(λ3z))+D4(C7sinh(λ4z)+C8cosh(λ4z)), (17) 式中:D1~D4为附加挠度系数,由恒等式原理确定,${D_i} = \dfrac{{E({I_{\rm{x}}} + {I_{{\rm{y}}{\text{ω}} }}){B_i}\lambda _i^3 - \bar m{\hat\omega ^2}}}{{\bar m{\hat\omega ^2} - E({I_{\text{ω}} } + {I_{{\rm{y}}{\text{ω}} }})\lambda _i^4 - G{A_{\text{ω}} }\lambda _i^2}} ,$${D_j} = \dfrac{{E({I_{\rm{x}}} + {I_{{\rm{y}}{\text{ω}} }}){B_j}\lambda _j^3 - \bar m{\hat\omega ^2}}}{{\bar m{\hat\omega ^2} - E({I_{\text{ω}} } + {I_{{\rm{y}}{\text{ω}} }})\lambda _j^4 + G{A_{\text{ω}} }\lambda _j^2}}$.
考虑双重效应影响的箱梁自振特性控制微分方程满足的边界条件为
简支端:${w_{\rm{s}}} = 0$,$f = 0$,${{ \dfrac{\partial \theta }{\partial {\textit{z}}} }} = 0$,${{ \dfrac{\partial^2 f }{\partial {\textit{z}^2} } }} = 0$;
固定端:${w_{\rm{s}}} = 0$,$ f = 0 $,$ \theta = 0 $,$ {{ \dfrac{\partial f}{\partial {\textit{z}} } }} = 0 $;
自由端:$\dfrac{\partial \theta }{\partial {\textit{z}}} = 0$,${{ \dfrac{\partial^2 f }{\partial {\textit{z}^2} } }} = 0$,${{ \dfrac{\partial w_{\rm{s}} }{\partial {\textit{z}} } }} - \theta = 0$,
∂3f∂z3+ζ∂2θ∂z2−k2∂f∂z=0. 1.2 考虑剪力滞影响的弯曲自振频率
当仅考虑剪力滞效应时,消除式(11)~(13)中的${{ \dfrac{\partial w }{\partial {\textit{z}} } }}$−θ项,然后将式中的θ替换为${{ \dfrac{\partial w }{\partial {\textit{z}} } }} $,即可得仅考虑剪力滞影响的箱梁自振频率控制微分方程:
ˉmˆω2w−EIx∂4w∂z4−EIyω∂4f∂z4=0, (18) ˉmˆω2f−EIω∂4f∂z4−EIyω∂4w∂z4+GAω∂2f∂z2=0. (19) 联立式(18)、(19),可得关于w的控制微分方程:
∂8w∂z8−J2∂6w∂z6−J7ˉmˆω2∂4w∂z4+J4ˉmˆω2∂2w∂z2+J6ˉm2ˆω4w=0, (20) 式中:${J_7} = \dfrac{{{I_{\rm{x}}} + {I_{\text{ω}} }}}{{E({I_{\rm{x}}}{I_{\text{ω}} } - I_{{\rm{y}}{\text{ω}} }^2)}}$.
求解式(20),可得自由振动时箱梁的初等梁挠度为
w0(z)=˜C1sin(˜λ1z)+˜C2cos(˜λ1z)+˜C3sin(˜λ2z)+˜C4cos(˜λ2z)+˜C5sinh(˜λ3z)+˜C6cosh(˜λ3z)+˜C7sinh(˜λ4z)+˜C8cosh(˜λ4z), (21) 式中:${\tilde C_1}$~${\tilde C_8}$为挠度待定系数,由边界条件确定;${\tilde \lambda _1}$ ~ ${\tilde \lambda _4}$为特征方程根.
联立式(18)、(19)得到关于附加挠度的微分方程,并将式(21)代入后可得
f(z)=˜D1(˜C1sin(˜λ1z)+˜C2cos(˜λ1z))+˜D2(˜C3sin(˜λ2z)+˜C4cos(˜λ2z))+˜D3(˜C5sinh(˜λ3z)+˜C6cosh(˜λ3z))+˜D4(˜C7sinh(˜λ4z)+˜C8cosh(˜λ4z)), (22) 式中:${\tilde D_1}$~${\tilde D_4}$为附加挠度系数,由恒等式原理确定,${\tilde D_i} = \dfrac{{E({I_{\rm{x}}} + {I_{{\rm{y}}{\text{ω}} }})\tilde \lambda _i^4 - \bar m{\hat\omega ^2}}}{{\bar m{\hat\omega ^2} - E({I_{\text{ω}} } + {I_{{\rm{y}}{\text{ω}} }})\tilde \lambda _i^4 - G{A_{\text{ω}} }\tilde \lambda _i^2}};$${\tilde D_j} = \dfrac{{E({I_{\rm{x}}} + {I_{{\rm{y}}{\text{ω}} }})\tilde \lambda _j^4 - \bar m{\hat\omega ^2}}}{{\bar m{\hat\omega ^2} - E({I_{\text{ω}} } + {I_{{\rm{y}}{\text{ω}} }})\tilde \lambda _j^4 + G{A_{\text{ω}} }\tilde \lambda _j^2}}$.
考虑剪力滞影响的箱梁自振特性控制微分方程满足的边界条件为
简支端:${w_0} = 0$,$ f = 0 $,${{ \dfrac{\partial^2 w }{\partial {\textit{z}^2} } }} = 0$,${{ \dfrac{\partial^2 f }{\partial {\textit{z}^2} } }} = 0$;
固定端:${w_0} = 0$,$ f = 0 $,${{ \dfrac{\partial w_0 }{\partial {\textit{z}} } }} = 0$,$ {{ \dfrac{\partial f }{\partial {\textit{z}} } }} = 0 $;
自由端:${{ \dfrac{\partial^2 w_0 }{\partial {\textit{z}^2} } }} = 0$,${{ \dfrac{\partial^2 f }{\partial {\textit{z}^2} } }} = 0$,${{ \dfrac{\partial^3 w_0 }{\partial {\textit{z}^3} } }}{\text{ = }}0$,
∂3f∂z3+ζ∂3w0∂z3−k2∂f∂z=0. 1.3 考虑剪切变形影响的弯曲自振频率
当仅考虑剪切变形时(即f = 0) ,式(11)~(13)进一步简化为
ˉmˆω2w+GAw(∂2w∂z2−∂θ∂z)=0, (23) EIx∂2θ∂z2+GAw(∂w∂z−θ)=0. (24) 联立式(23)、(24),可得关于w的控制微分方程:
∂4w∂z4+ˉmˆω2GAw∂2w∂z2−ˉmˆω2EIxw=0. (25) 求解式(25),可得自由振动时考虑剪切变形影响的箱梁挠度ws(z)为
ws(z)=ˉC1sin(ˉλ1z)+ˉC2cos(ˉλ1z)+ˉC3sinh(ˉλ2z)+ˉC4cosh(ˉλ2z), (26) 式中:${\bar C_1}$~${\bar C_4}$为挠度待定系数,由边界条件确定;${\bar \lambda _1}$、${\bar \lambda _2}$为特征方程根.
根据挠度和转角的微分关系,可得转角θ(z)为
θ(z)=ˉB1(ˉC1cos(ˉλ1z)−ˉC2sin(ˉλ1z))+ˉB2×(ˉC3cosh(ˉλ2z)+ˉC4sinh(ˉλ2z)), (27) 式中:${\bar B_1}$、${\bar B_2}$为截面转角待定系数,由恒等式原理确定,即${\bar B_i} = {\bar \lambda _i}\left(1 - \dfrac{{\bar m{\hat\omega ^2}}}{{G{A_{\rm{w}}}\bar \lambda _i^2}}\right)$.
考虑剪切影响的箱梁自振特性控制微分方程满足的边界条件为
简支端:${w_{\rm{s}}} = 0$,$ {{ \dfrac{\partial \theta }{\partial {\textit{z}} } }} = 0 $;
固定端:${w_{\rm{s}}} = 0$,$ \theta = 0 $;
自由端:${{ \dfrac{\partial \theta }{\partial {\textit{z}} } }}= 0 $,${{ \dfrac{\partial w_{\rm{s}} }{\partial {\textit{z}} } }} - \theta = 0$.
2. 弯曲自振频率求解
2.1 简支箱梁
本节建立考虑剪力滞、剪切及双重效应影响的箱梁弯曲自振频率fsl、fsd和fss. 根据考虑双重效应影响的8个边界条件,可得关于C1~C8的8个线性方程,欲使该方程组有非零解,则该系数行列式为0,即sin (λ1l)=0,其中,λ1=nπ/l (n为频率阶数,n=1,2,3,…).
将式(14)对应的特征方程根${r_1} = {\lambda _1}{\rm{i}}$代入特征方程后,可得考虑双重效应影响的简支箱梁自振圆频率ωss,根据fo=$\hat\omega_o $/(2π) (o∈{sl,sd,ss})可得
fss=ass2π (nπl)2√EIxˉm, (28) 式中:ass为考虑双重效应影响的弯曲自振频率修正系数,$a{}_{{\rm{ss}}}{\text{ }} = \dfrac{{\text{1}}}{{{\text{2}}\left( {\lambda _{\text{1}}^{\text{2}}{J_5} + {J_6}} \right)}} \sqrt {\dfrac{{ - 2}}{{E{I_x}}}\left( {{J_5} + \dfrac{{{J_6}}}{{\lambda _{\text{1}}^{\text{2}}}}} \right)\left( {J - {J_1}\lambda _{\text{1}}^{\text{4}} - {J_3}\lambda _{\text{1}}^{\text{2}} - {J_4}} \right)}$,J=$\left[ \lambda _1^8J_1^2 \,+\, 2\lambda _1^6\left( {{J_1}{J_3} \,-\, 2{J_5}} \right) \,+\, \lambda _1^4(2{J_1}{J_4} - 4{J_2}{J_5} + J_3^2 - 4{J_6}) -\right.$ $\left.{2\lambda _1^2\left( {2{J_2}{J_6} - {J_3}{J_4}} \right) + J_4^2}\right] ^{\tfrac{1}{2}}.$
同理可得,考虑剪力滞影响的箱梁弯曲自振频率为
fsl=asl2π (nπl)2√EIxˉm, (29) 式中:asl为考虑剪力滞影响的弯曲自振频率修正系数,$a{}_{{\rm{sl}}}{\text{ }} = \dfrac{{\text{1}}}{{\sqrt {2{J_6}E{I_{\rm{x}}}} }}\sqrt {{J_7} - \sqrt {J_7^2 - 4{J_6} - \dfrac{{4{J_2}{J_6}}}{{\tilde \lambda _{\text{1}}^{\text{2}}}} + \dfrac{{2{J_4}{J_7}}}{{\tilde \lambda _{\text{1}}^{\text{2}}}} + \dfrac{{J_4^2}}{{\tilde \lambda _{\text{1}}^4}}} + \dfrac{{{J_4}}}{{\tilde \lambda _{\text{1}}^{\text{2}}}}}$,
${\tilde \lambda _1}$=nπ/l.
同理可得考虑剪切影响的箱梁弯曲自振频率为
fsd=asd2π (nπl)2√EIxˉm, (30) 式中:asd为考虑剪切影响的简支箱梁弯曲自振频率修正系数,即$a{}_{{\rm{sd}}} = \sqrt {\dfrac{{G{A_{\rm{w}}}}}{{G{A_{\rm{w}}} + E{I_x}\bar \lambda _1^2}}} $,${\bar \lambda _1}$=nπ/l.
上述建立了考虑剪力滞、剪切及双重效应影响的简支箱梁弯曲自振频率计算式,均基于初等梁理论进行了修正,当修正系数为1时,则退化为初等梁弯曲自振频率f0.
2.2 连续箱梁
将上节简支梁弯曲自振频率计算方法运用至连续箱梁中,会出现参数多、方程数量多、求解麻烦等问题. 为简化连续箱梁自振频率的计算过程,本节将采用三弯矩法[11-12]进行求解. 实际工程中多跨连续箱梁主要采用不等跨的布置形式,而边中跨径比是影响连续箱梁弯曲自振频率的主要因素之一[12]. 设第一跨跨径和最后一跨跨径分别为l1和lm,且l1=lm=lb,中跨跨径l2=l3=…=lm−1=l,不等跨连续箱梁自由振动的变形协调方程[11-12]为
[Gm+GbHm000⋯0000Hm2GmHm00⋯00000Hm2GmHm0⋯0000⋮⋮⋮⋮⋮⋮⋮⋮⋮00000⋯0Hm2GmHm00000⋯00HmGm+Gb]×[M1M2M3⋯Mm−1Mm]T=0, (31) 式中:Gm=cot(λl)−coth(λl),Gb=cot(λlb)−coth(λlb),Hm=1/sinh(λl)−1/sin(λl),λ为连续梁频率参数,Mm为中支点处的弯矩.
由式(31)可知,该方程组有非零解和零解2种情况. 非零解对应系数行列式为0,此时结构存在正、反对称2种振型;零解对应各中支点弯矩为0,可将其当做若干个简支梁进行求解. 为研究方便,此处定义频率参数λi=$\xi $π/l,$\xi $为频率影响因子. 以三跨连续梁为例,下面直接列出后续连续梁计算所需的$ \xi $值,如表1所示.
表 1 不等跨连续梁自振频率参数影响因子Table 1. Influence factors of natural vibration frequency parameters of continuous girders with unequal spanlb/l n 1 2 3 4 5 6 0.5 1.250 2.000 2.250 2.500 3.250 4.000 0.6 1.215 1.777 1.926 2.340 3.137 3.503 0.7 1.174 1.565 1.699 2.264 2.908 3.060 0.8 1.125 1.390 1.542 2.200 2.613 2.728 0.9 1.066 1.248 1.437 2.118 2.351 2.500 1.0 1.000 1.132 1.368 2.000 2.135 2.365 将简支箱梁自振频率表达式(式(28)~(30))中的n值替换为$\xi $,即可得到连续箱梁考虑剪力滞、剪切及双重效应影响的弯曲自振频率计算式.
3. 算例分析
3.1 简支箱梁
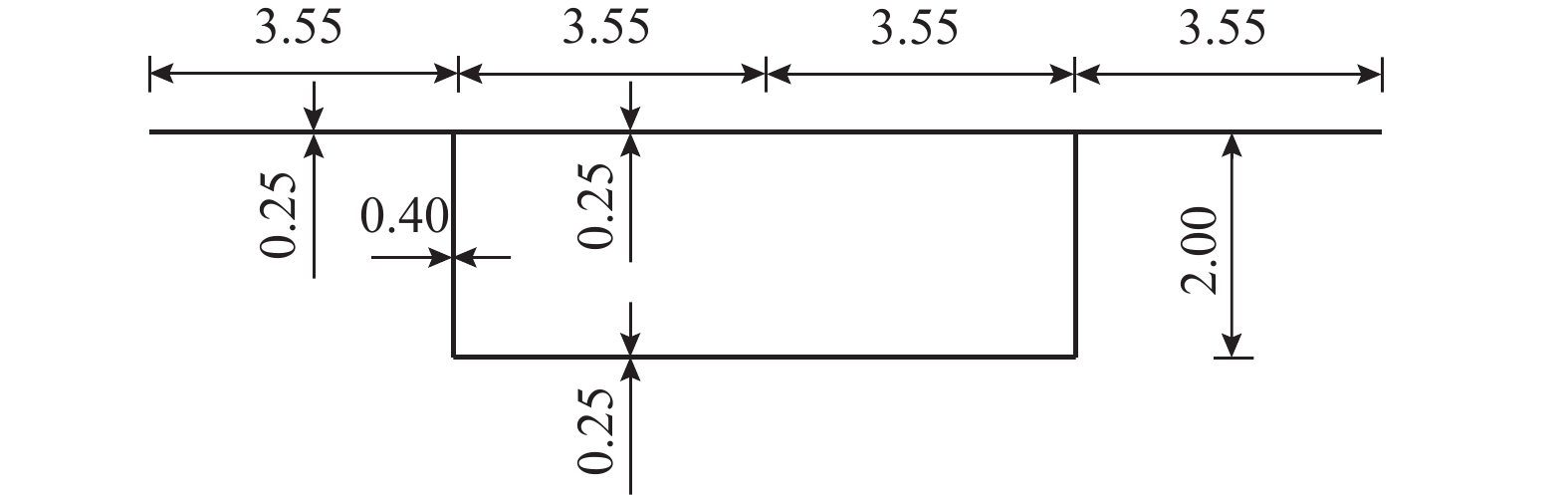
以文献[19]介绍的简支箱梁为例,截面尺寸如图2所示,跨度l=40 m,b1=b2=b3=3.55 m,tu=tb= 0.25 m,tw= 0.40 m,E=35 GPa,泊松比μ=0.166 7,G=15 GPa,密度ρ=2 500 kg/m3.
利用ANSYS-shell63单元建立简支箱梁空间有限元模型(共划分
4626 个节点,4608 个单元),除梁端约束外,其余节点均施加节点横向自由度(Ux)、绕y轴的自由度(Ry)、绕z轴的自由度(Rz) 3个约束,分析并计算考虑剪切剪力滞影响的箱梁弯曲自振频率;利用Midas-civil建立箱梁杆系有限元模型(共划分41个节点,40个单元),其约束方式与上述ANSYS模型一致,计算得到考虑截面剪切影响的箱梁弯曲自振频率;采用本文方法计算得到考虑各效应影响的简支箱梁前6阶弯曲自振频率,并连同ANSYS壳单元计算结果和Midas杆系有限元结果一并列入表2,以便对比.表 2 简支箱梁弯曲自振频率对比Table 2. Comparison of bending natural vibration frequency of simply supported box girderHz n f0 fsl fsd fss ANSYS 结果 Midas 结果 1 3.243 3.160 3.167 3.090 3.108 3.121 2 12.973 11.663 11.871 10.874 10.783 11.124 3 29.188 23.250 24.349 20.851 22.957 23.385 4 51.890 36.511 38.927 31.891 33.325 33.891 5 81.079 51.398 54.491 43.838 44.681 45.332 6 116.753 68.215 70.429 56.690 59.690 60.449 从表2可以看出:考虑剪切和双重效应影响的弯曲自振频率解析解与有限元数值解吻合程度较好,验证了本文方法的正确性;剪力滞和剪切效应对自振频率的削弱程度相当,其原因在于本算例中箱室较宽,剪力滞效应较为突出而导致的;单个效应和双重效应对自振频率的影响不满足线性叠加原理;各效应对低阶频率的影响较小,而对高阶频率影响较为显著;对于一阶频率而言,剪力滞、剪切和双重效应对初等梁自振频率分别削弱了2.56%、2.34%和4.72%.
3.2 参数敏感性分析
为分析截面参数对各效应下弯曲自振频率的削弱程度,定义频率差值比参数为
Δfo = f0−fof0×100%. (32) 以3.1节给出的简支箱梁算例为基础,详细分析跨宽比(l/(2b1))、宽高比(2b1/h)、板宽比(b3/b1)对箱梁弯曲自振频率差值比的影响.
1) 跨宽比(l/(2b1))影响分析
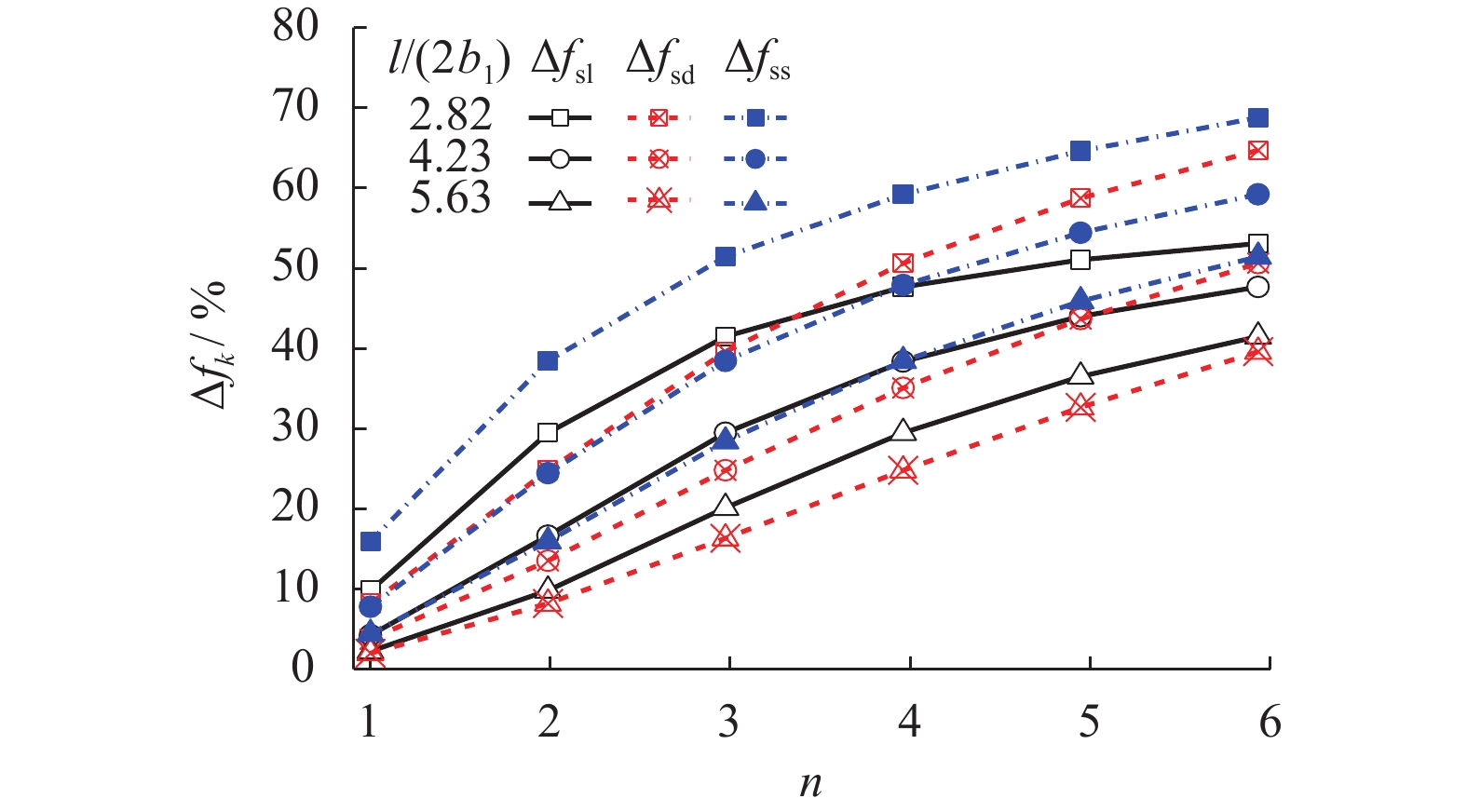
在上节简支箱梁算例基础上,仅改变跨度,其他尺寸保持不变. 当跨度取20、30、40 m时,对应跨宽比l/(2b1)=2.82,4.23,5.63. 运用本文解析法计算并绘制各效应影响下箱梁弯曲自振频率差值比分布曲线,如图3所示.
由图3可以看出:跨宽比越大,自振频率差值比越小,即各效应对初等梁自振频率的削弱程度随之减小;频率阶数越大,各效应对初等梁自振频率的削弱程度越大;同一跨宽比下,前三阶频率,剪力滞削弱影响略大于剪切效应,后三阶频率,随着跨宽比的减小,剪切效应影响大于剪力滞;同一跨宽比时,剪力滞和剪切效应对自振频率的削弱程度相当.
2) 宽高比(2b1/h)影响分析
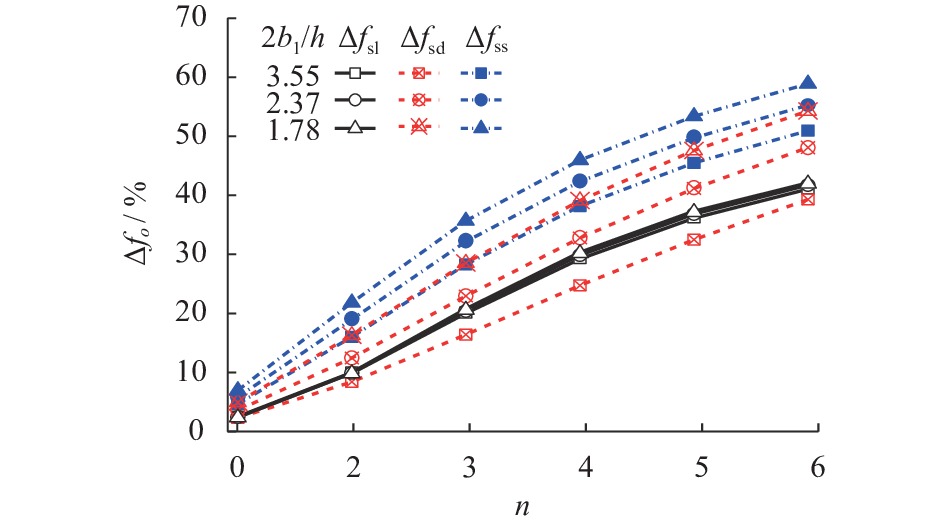
在上节简支箱梁算例基础上,改变梁高,截面其他尺寸及跨度保持不变;当梁高取2.0、3.0、4.0 m时,对应的宽高比2b1/h =3.55,2.37,1.78;运用本文解析法计算并绘制各效应影响的箱梁弯曲自振频率差值比分布曲线,如图4所示.
由图4可以看出:宽高比越大,自振频率差值比越小,即各效应对初等梁自振频率的削弱程度逐渐减小;频率阶数越大,各效应对初等梁自振频率的削弱程度越大;宽高比对剪切自振频率差值比影响较大,双重效应自振频率差值比影响次之,剪力滞自振频率差值比影响甚小,说明不同宽高比下,剪力滞效应对自振频率的削弱程度近乎相同.
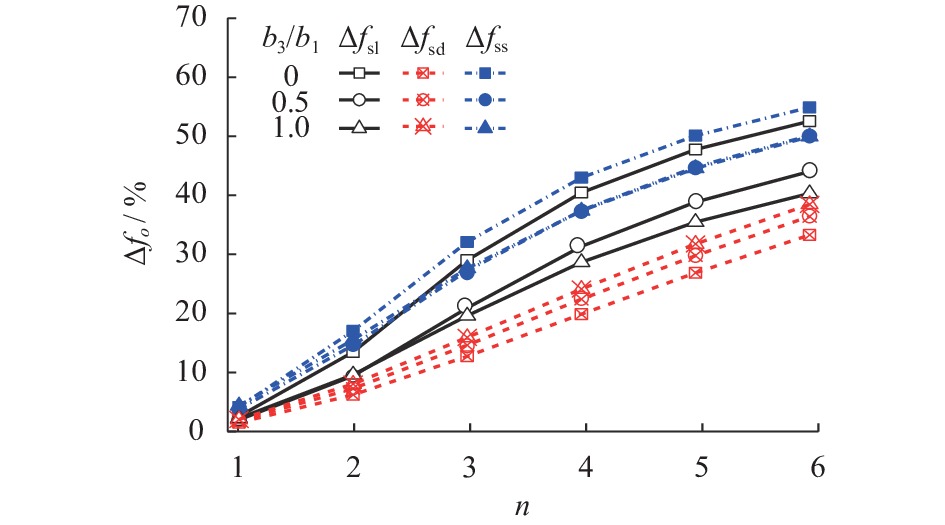
3) 板宽比(b3/b1)影响分析
在上节简支箱梁算例基础上,改变悬臂板宽度,截面其他尺寸及跨度不变. 顶板半宽b1=3.55 m,取悬臂板宽度b3=0,1.775,3.55 m,其对应的板宽比b3/b1=0,0.5,1.0,运用本文解析法计算并绘制各效应影响的箱梁弯曲自振频率差值比分布曲线,如图5所示. 由图可以看出:板宽比越大,剪力滞、双重效应自振频率差值比越小,剪切自振频率差值比越大;频率阶数越大,各效应对初等梁自振频率的削弱程度越大;板宽比由0增大至0.5时,剪力滞效应对自振频率的削弱程度较显著;不同板宽比下,剪切和双重效应对自振频率的削弱程度相当;低阶自振频率计算时可按不带悬臂板的箱梁进行计算,高阶频率计算需要考虑悬臂板的影响.
3.3 连续箱梁
以3.1节介绍的简支箱梁为例,截面尺寸、材料特性(弹性模量、剪切模量、密度等)不变,将其变为3等跨连续箱梁,即l1=l2=l3=40 m.
利用ANSYS-shell63单元建立箱梁空间有限元模型(共划分
13676 个节点,13696 个单元),除梁端约束条件外,其余节点采用3.1节中的约束方式,分析并计算考虑剪切剪力滞效应影响的连续箱梁弯曲自振频率. 同时,利用Midas-civil建立杆系有限元模型(共划分121个节点,120个单元),计算得到考虑截面剪切影响的连续箱梁弯曲自振频率. 采用本文方法计算得到考虑各效应影响的连续箱梁前6阶弯曲自振频率,并连同ANSYS壳单元计算结果和Midas杆系有限元结果列入表3,以便对比.表 3 连续箱梁弯曲自振频率对比Table 3. Comparison of bending natural vibration frequency of continuous box girderHz n f0 fsl fsd fss ANSYS 结果 Midas 结果 1 3.246 3.160 3.170 3.090 3.192 3.221 2 4.157 4.019 4.033 3.908 4.036 4.242 3 6.073 5.778 5.815 5.556 5.356 5.667 4 12.978 11.663 11.875 10.874 10.048 10.524 5 14.794 13.097 13.385 12.135 11.877 12.487 6 18.150 15.648 16.093 14.356 12.366 13.191 从表3可以看出:考虑剪切和双重效应影响的箱梁弯曲自振频率解析解与有限元数值解吻合较好,验证了本文方法的正确性;与简支箱梁相比,中跨跨径等于简支跨径的3跨连续箱梁一阶频率差值比甚小,二阶后的频率差值比较大;对于一阶频率而言,剪力滞、剪切和双重效应对初等梁自振频率分别削弱了2.64%、2.35%和4.80%.
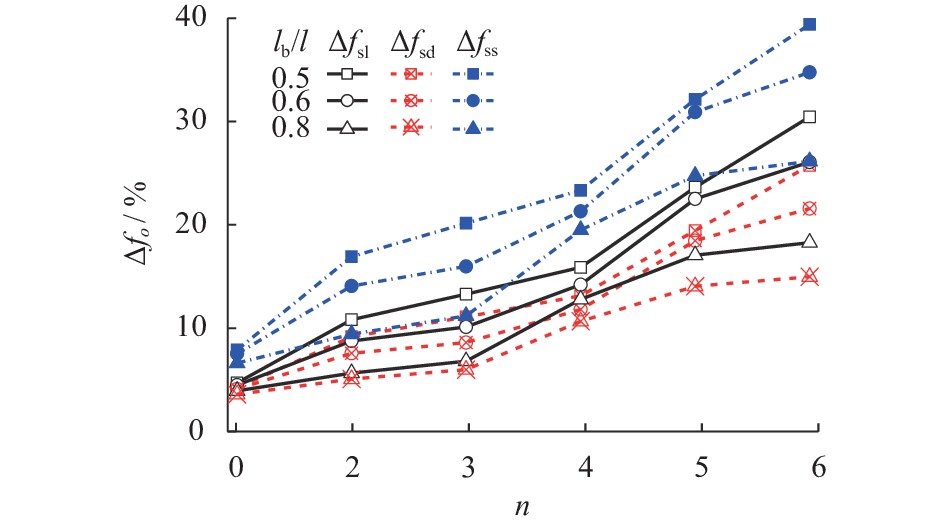
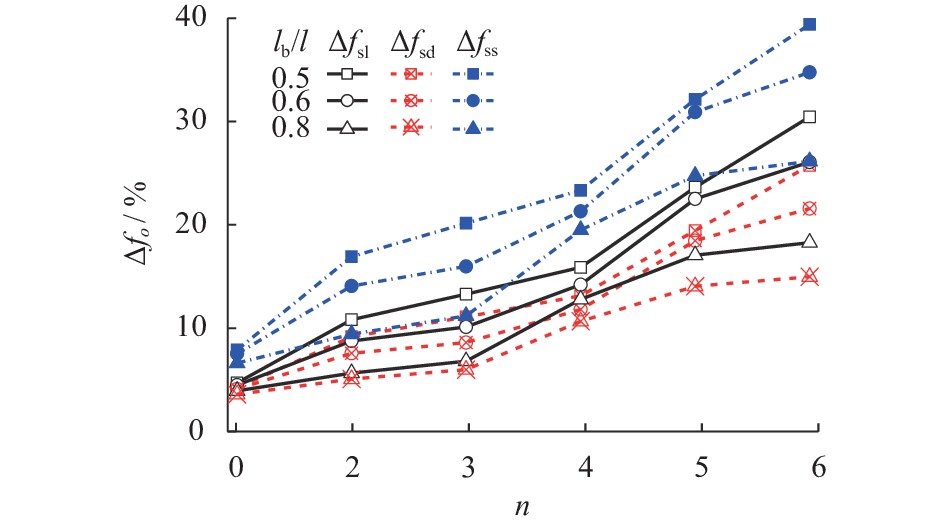
边中跨径比是影响箱梁弯曲自振频率的一个主要因素,下面基于本节介绍的3等跨连续箱梁为例,保持截面参数及材料特性不变,仅改变边跨跨径. 当边跨跨长lb=20,24,32 m,对应lb/l=0.5,0.6,0.8,运用本文方法计算并绘制不同边中跨径比下考虑剪力滞、剪切和双重效应影响的连续箱梁弯曲自振频率差值比分布图,如图6所示.
由图6可以看出:边中跨径比越大,自振频率差值比越小,即各效应对连续箱梁初等梁自振频率的削弱程度逐渐减小;频率阶数越大,各效应对初等梁自振频率的削弱程度越大;剪力滞效应对自振频率的削弱影响略大于剪切效应.
4. 结 论
本文以剪力滞附加挠度为广义位移,并将其纳入箱梁总动能中,运用Hamilton原理建立了考虑剪切、剪力滞及双重效应影响的箱梁弯曲自振频率变分解,结合数值算例得到以下主要结论:
1) 考虑剪切和双重效应影响的弯曲自振频率解析解与有限元数值解吻合较好;单个效应和双重效应对自振频率的影响不满足线性叠加原理;各效应对低阶频率影响较小,对高阶频率影响较大,其中双重效应影响最为显著,对一阶频率,双重效应对简支和连续箱梁自振频率分别削弱了4.72%和4.80%.
2) 简支箱梁参数分析表明,跨宽比、宽高比越大,自振频率差值比越小,即各效应对自振频率的削弱程度越小;同一跨宽比时,剪力滞和剪切效应对自振频率的削弱程度相当;宽高比对剪切自振频率差值比影响较大,剪力滞自振频率差值比影响甚小;板宽比越大,剪力滞、双重效应自振频率差值比越小,剪切自振频率差值比越大;低阶自振频率计算时可按不带悬臂板的箱梁进行计算.
3) 连续箱梁参数分析表明,边中跨径比越大,自振频率差值比越小,即各效应对连续箱梁自振频率的削弱程度越小;各效应对三跨连续箱梁(中跨跨径等于简支跨径)自振频率的削弱影响远小于简支箱梁.
-
表 1 不等跨连续梁自振频率参数影响因子
Table 1. Influence factors of natural vibration frequency parameters of continuous girders with unequal span
lb/l n 1 2 3 4 5 6 0.5 1.250 2.000 2.250 2.500 3.250 4.000 0.6 1.215 1.777 1.926 2.340 3.137 3.503 0.7 1.174 1.565 1.699 2.264 2.908 3.060 0.8 1.125 1.390 1.542 2.200 2.613 2.728 0.9 1.066 1.248 1.437 2.118 2.351 2.500 1.0 1.000 1.132 1.368 2.000 2.135 2.365 表 2 简支箱梁弯曲自振频率对比
Table 2. Comparison of bending natural vibration frequency of simply supported box girder
Hz n f0 fsl fsd fss ANSYS 结果 Midas 结果 1 3.243 3.160 3.167 3.090 3.108 3.121 2 12.973 11.663 11.871 10.874 10.783 11.124 3 29.188 23.250 24.349 20.851 22.957 23.385 4 51.890 36.511 38.927 31.891 33.325 33.891 5 81.079 51.398 54.491 43.838 44.681 45.332 6 116.753 68.215 70.429 56.690 59.690 60.449 表 3 连续箱梁弯曲自振频率对比
Table 3. Comparison of bending natural vibration frequency of continuous box girder
Hz n f0 fsl fsd fss ANSYS 结果 Midas 结果 1 3.246 3.160 3.170 3.090 3.192 3.221 2 4.157 4.019 4.033 3.908 4.036 4.242 3 6.073 5.778 5.815 5.556 5.356 5.667 4 12.978 11.663 11.875 10.874 10.048 10.524 5 14.794 13.097 13.385 12.135 11.877 12.487 6 18.150 15.648 16.093 14.356 12.366 13.191 -
[1] 《中国公路学报》编辑部. 中国桥梁工程学术研究综述·2021[J]. 中国公路学报, 2021, 34 (2): 1-97.Editorial Department of China Journal of Highway and Transpor. Review on China’s bridge engineering research: 2021[J]. China Journal of Highway and Transport, 2021, 34 (2): 1-97. [2] 冀伟,罗奎,马万良,等. 装配式波形腹板钢箱-混凝土组合梁桥动力特性分析与试验研究[J]. 振动与冲击,2020,39(20): 1-7,16.JI Wei, LUO Kui, MA Wanliang, et al. Dynamic characteristics analysis and experimental study of a fabricated corrugated web steel box-concrete composite girder bridge[J]. Journal of Vibration and Shock, 2020, 39(20): 1-7,16. [3] DOMAGALSKI Ł. Comparison of the natural vibration frequencies of Timoshenko and Bernoulli periodic beams[J]. Materials, 2021, 14(24): 7628.1-7628.22. [4] JI W, LUO K, MA W L. Natural vibration frequency analysis for a PC continuous box-girder bridge with corrugated steel web based on the dynamic stiffness matrix[J]. Journal of Highway and Transportation Research and Development (English Edition), 2020, 14(1): 65-74. doi: 10.1061/JHTRCQ.0000718 [5] SUN Q K, ZHANG N, LIU X. A dynamic stiffness matrix method for free vibrations of partial-interaction composite beams based on the Timoshenko beam theory[J]. Journal of Sound and Vibration, 2022, 520: 116579.1-116579.14. [6] JIANG L Z, LAI Z P, ZHOU W B. Improved finite beam element method for analyzing the flexural natural vibration of thin-walled box girders[J]. Advances in Mechanical Engineering, 2017, 9(8): 1888-1902. [7] 房建,韦智敏,郑稳稳,等. 城市高架轨道交通引发的箱梁振动特性分析[J]. 铁道学报,2022,44(4): 136-142.FANG Jian, WEI Zhimin, ZHENG Wenwen, et al. Study on vibration characteristics of elevated box girders induced by track irregularities[J]. Journal of the China Railway Society, 2022, 44(4): 136-142. [8] 张新亚,雷晓燕,罗锟. TMD控制高架轨道箱梁结构振动的模型试验研究[J]. 振动与冲击,2021,40(16): 220-226,233.ZHANG Xinya, LEI Xiaoyan, LUO Kun. A model test study on controlling vibration of an elevated track box girder structure with TMD[J]. Journal of Vibration and Shock, 2021, 40(16): 220-226,233. [9] 郑尚敏,万水,程海根. 单箱多室波形钢腹板组合箱梁动力特性研究[J]. 铁道工程学报,2017,34(9): 41-46.ZHENG Shangmin, WAN Shui, CHENG Haigen. Research on the dynamic characteristics of multi-room single box composite girder with corrugated steel webs[J]. Journal of Railway Engineering Society, 2017, 34(9): 41-46. [10] JIANG L Z, YU J, ZHOU W B, et al. Analysis of flexural natural vibrations of thin-walled box beams using higher order beam theory[J]. The Structural Design of Tall and Special Buildings, 2019, 28(14): 1659.1-1659.15. [11] 李丽园,周茂定,张元海. 薄壁连续箱梁的弯曲自振频率分析[J]. 东南大学学报(自然科学版),2018,48(1): 99-105.LI Liyuan, ZHOU Maoding, ZHANG Yuanhai. Analysis of bending natural vibration frequency of thin-walled continuous box girder[J]. Journal of Southeast University (Natural Science Edition), 2018, 48(1): 99-105. [12] 周茂定,蔺鹏臻,李丽园. 考虑各板面内剪切影响的箱梁竖向纯弯自振特性分析[J]. 振动工程学报,2020,33(6): 1162-1169.ZHOU Maoding, LIN Pengzhen, LI Liyuan. Analysis of vertical pure bending natural vibration characteristics of box girder considering the influence of in-plane shear[J]. Journal of Vibration Engineering, 2020, 33(6): 1162-1169. [13] ZHANG Y T, JIANG L Z, ZHOU W B, et al. Shear lag effect and accordion effect on dynamic characteristics of composite box girder bridge with corrugated steel webs[J]. Applied Sciences, 2020, 10(12): 4346.1-4346.13. [14] 项贻强,邱政,何百达,等. 具有体外预应力索的快速施工群钉式钢-混组合小箱梁自振特性分析[J]. 中国公路学报,2020,33(1): 100-110.XIANG Yiqiang, QIU Zheng, HE Baida, et al. Analytical approach for the free vibration of accelerated construction steel-concrete composite small box girders with external prestressed tendons and group studs[J]. China Journal of Highway and Transport, 2020, 33(1): 100-110. [15] SHEN J, PAGANI A, ARRUDA M R T, et al. Exact component-wise solutions for 3D free vibration and stress analysis of hybrid steel-concrete composite beams[J]. Thin-Walled Structures, 2022, 174: 109094.1-109094.13. [16] 冀伟,温凯康,罗奎. 基于Galerkin法的新型波形钢腹板箱梁桥动力特性研究[J]. 工程科学与技术,2022,54(3): 131-138.JI Wei, WEN Kaikang, LUO Kui. Dynamic characteristics of new type composite box girder bridge with corrugated steel webs based on Galerkin method[J]. Advanced Engineering Sciences, 2022, 54(3): 131-138. [17] CAI Y X, ZHANG K, YE Z J, et al. Influence of temperature on the natural vibration characteristics of simply supported reinforced concrete beam[J]. Sensors, 2021, 21(12): 4242.1-4242.14. [18] QIU C, XIE X L, YANG C J, et al. Methods of improving the natural vibration characteristics of the through tied-arch bridge and test verification[J]. International Journal of Steel Structures, 2022, 22(1): 343-360. doi: 10.1007/s13296-022-00577-3 [19] 张玉元. 考虑翼板横向位移影响的箱梁弯曲静动力特性分析理论及其应用研究[D]. 兰州: 兰州交通大学, 2020. -






 下载:
下载:





 下载:
下载: