Simplified Calculation Method for Dynamic Characteristics of Pulse Wind Tunnel Balance Foundation
-
摘要:
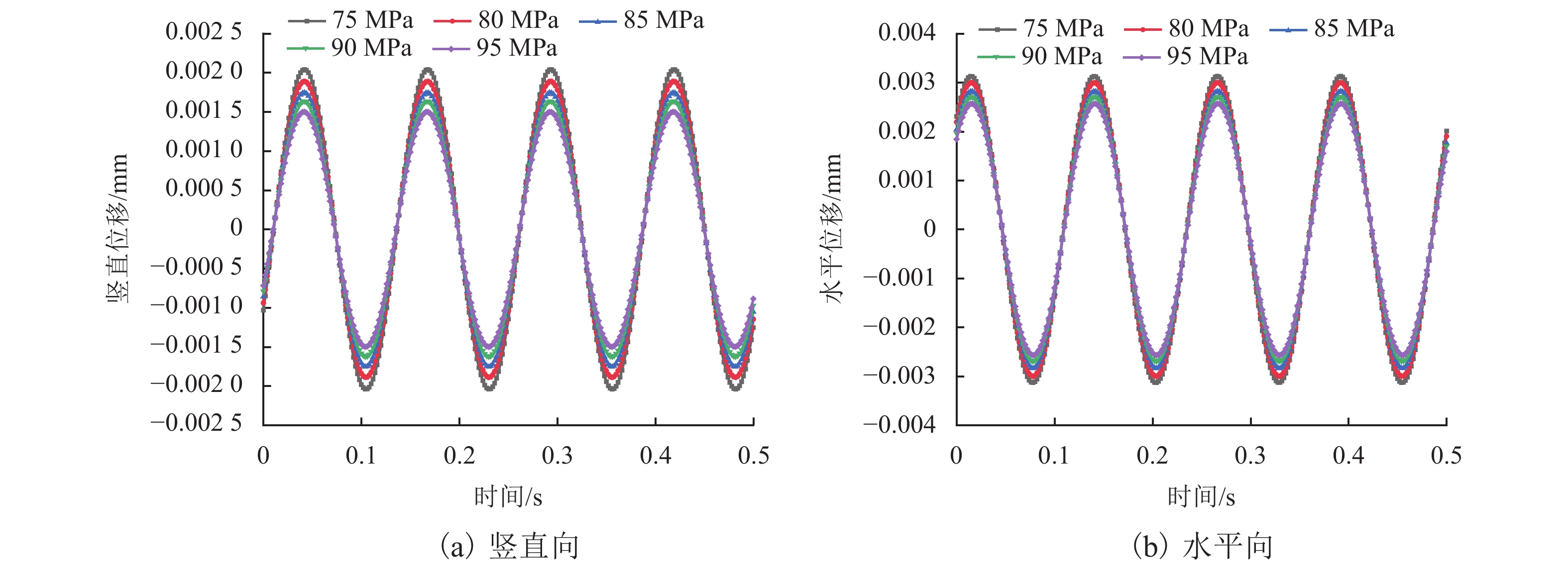
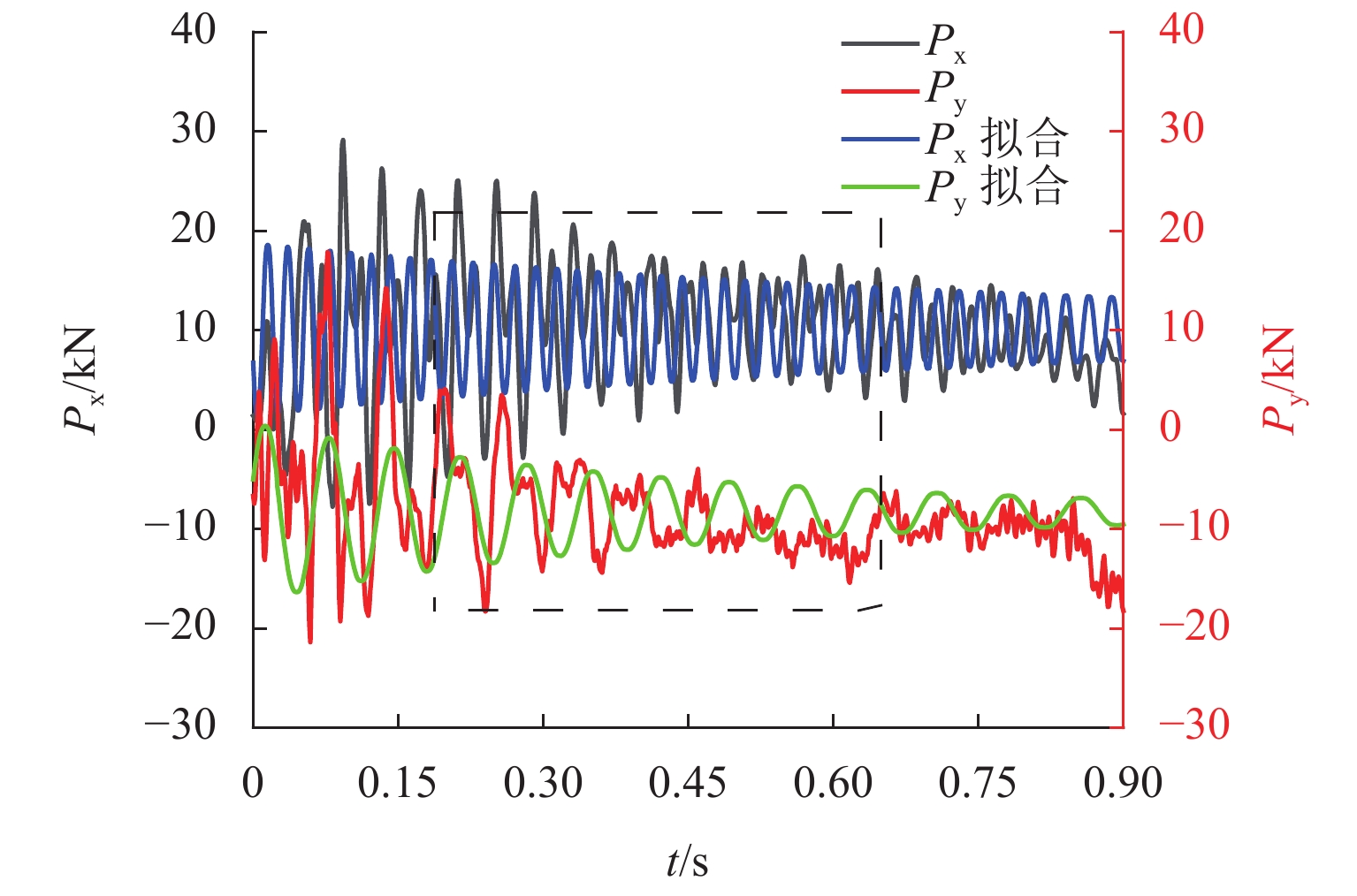
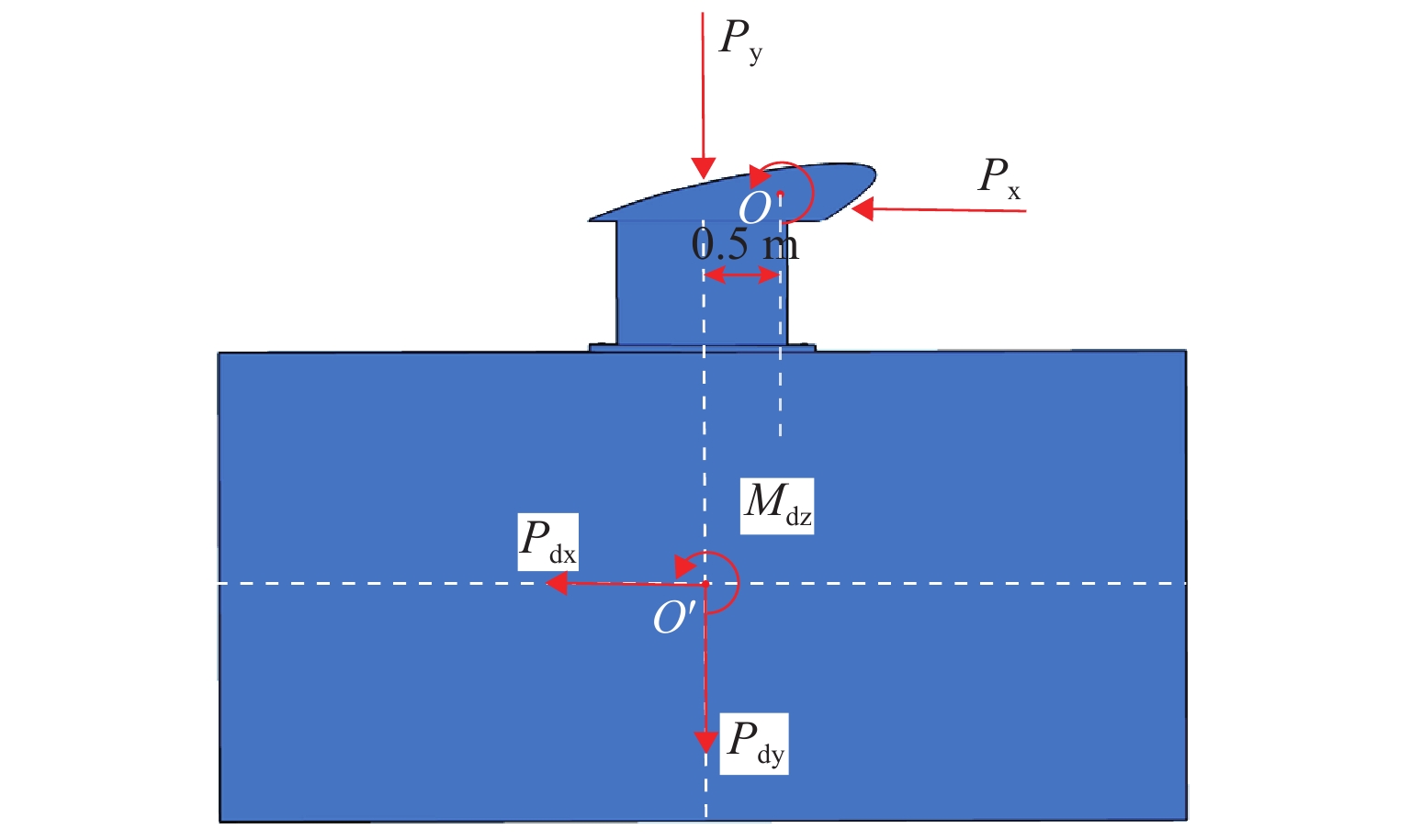
为了研究脉冲风洞天平基础在脉冲气动荷载作用下的动力响应特征,以某脉冲风洞为例,选择某典型气动荷载作用形式,建立了脉冲风洞天平基础在气动荷载作用下竖直方向、水平方向位移以及回转角度等动力特征的简化计算方法,并采用数值模拟的方法验证其可靠性. 研究结果表明:天平基础在典型气动荷载作用下,竖直向最大振幅0.00175 mm,频率7.94 Hz,水平向最大振幅0.00283 mm,频率7.94 Hz,回转角度最大振幅0.00034°,频率7.94 Hz,典型气动荷载对天平基础振动影响较小,也未产生共振现象;基础振动最大振幅随气动荷载增大而增大,基础振动频率随气动荷载频率增大而增大;在气动荷载不变的条件下,基础振动的最大振幅与频率随尺寸的变大而变小,基础振动的最大振幅也随地基土性质的增强而减小,但地基土性质的变化对基础振动频率无影响.
Abstract:In order to study the dynamic response characteristics of the pulse wind tunnel balance foundation under the action of pulse aerodynamic loads, a pulse wind tunnel was taken as an example, and a typical aerodynamic load action type was selected. As a result, a simplified calculation method for dynamic characteristics of the pulse wind tunnel balance foundation such as displacement of the vertical direction, displacement of the horizontal direction, and rotation angle was established, and its reliability was verified by numerical simulation. The results show that under the action of a typical aerodynamic load, the balance foundation has a maximum vertical vibration amplitude of 0.001 75 mm and a frequency of 7.94 Hz; a maximum horizontal vibration amplitude of 0.002 83 mm and a frequency of 7.94 Hz; a maximum amplitude of the rotation angle of 0.000 34° and a frequency of 7.94 Hz. The typical aerodynamic load has little effect on the vibration of the balance foundation, and no resonance phenomenon occurs. Meanwhile, the maximum amplitude of foundation vibration increases with the increase in aerodynamic load, and the frequency of foundation vibration increases with the increase in aerodynamic load frequency; under the condition of constant aerodynamic load, the maximum amplitude and frequency of foundation vibration gradually decrease with the increase in size; the maximum amplitude of the foundation vibration decreases with the increase in foundation soil properties, but the change of foundation soil properties has no effect on the foundation vibration frequency.
-
气候恶化与能源危机的双重压力下,以光伏发电为主的可再生能源利用形式取代传统燃煤、燃油发电等化石能源消耗,已日益成为世界范围能源结构转型的主要发展方向. 预计至2050年,全球光伏发电总量将占据整体电力构成的25%左右[1],而我国更是提出至2050年光伏发电量占全社会用电总量39%的总体发展目标[2],远高于世界平均发展预期. 其主要原因在于我国光伏组件成本的快速下降及日趋成熟的商业运作模式,但如此快速的发展进程也对未来光伏发电的整体空间部署提出新的要求. 利用戈壁、荒漠等光资源较好的未利用土地集中化部署光伏电站,具有电力输出更为平稳可控及度电成本相对低廉(规模经济效应)等明显优势,但相比城市分布式光伏利用,光伏电站通常依赖长距离电力调配,产生输电损失的同时,还将增加额外输配系统建设投入;此外,其使用周期较长(约25年)且空间占用面积较大,由此所导致的土壤、植被破坏及物种多样性缺失等生态环境影响也同样不容忽视[3-5]. 因此,选择适宜区域进行光伏电站建设,对于合理指导光伏电站有序开发、保障能源结构可持续转型具有重要意义.
光伏电站选址工作具备一定的复杂性,其站点位置决策通常与电站安全、气象需要、经济、环境与社会等需求密切相关[6]. 为同时兼顾经济与生态两方面收益,目前,国际范围针对光伏电站选址收益的评价多采用以地理信息系统(GIS)与各项评价指标相结合的多准则决策法(MCDM)进行判定. 该方法以层次分析法(AHP)为基础理论依据,按照电站发展需要制定相关影响因素决策性指标(如日照辐射量、距输电线距离、地形条件等),通过专家打分形式对每项决策性指标所占权重作出评估并进行加权,之后利用GIS空间分析对每处地块区域的指标加权结果进行总分统计,生成光伏利用适宜性地图,以此确立开发建设优先级. 目前,该方法已广泛应用于世界范围不同地区的光伏选址收益研究[7-10],通过各地块加权总分排序,可在一定程度为光伏电站建设布局提供规划指导,其相关影响因素分析也为光伏电站选址收益评估提供了重要的理论支撑. 但基于AHP的固有缺陷,其本质仍是一个定性研究的过程,其中的主观性因素难以排除. 此外,既有方法也无法对电站实际生态经济收益量、增减益等问题做出直观的量化描述,因此,其实际指导意义仍存在诸多局限.
出于以上考虑,本文提出一种以能值理论为基础的光伏电站选址及生态经济收益评估方法,将不同影响因素视作光伏电站系统的各项能值投入,通过能值转换率(UEV)将其转化为以太阳能值为标准的统一度量维度,并以能值产投比(EROI)为指标,对各地块区域进行光伏装机后的生态经济收益情况做出评估,就各项能值投入所占比重展开分析,进而为光伏电站有序开发、能源空间整体布局规划提供更为科学的理论指导及参考依据.
1. 研究方法
1.1 基础指标
能值理论最早由美国生态学家Odum[11]提出. 基于生态系统食物链概念和热力学原理,能值被定义为一种流动或储存的能量中包含另一种类别能量的数量,或产品及劳动形成的过程中直接或间接投入应用的一种有效能的总量. 由于任何能量均源自太阳能,故通常以太阳能值作为衡量任何类别能量的标准,并计作太阳能焦耳(单位:sej),单位能量或物质所包含太阳能值称作其能值转换率[12]. 与MCDM方法不同,能值评估并非基于人类价值体系的主观因素评估自然界对经济活动的贡献率,而是一种以生态价值为中心,更具客观性及真实性的生物物理学方法[13]. 因此,以能值理论为依据能够有效实现不同度量维度影响因素间的相互转换,通过模拟及对比光伏电站建设后的能值产出与能值投入情况,即能值产投比(EROI,EROI),可更为科学地反映光伏发电系统结构、功能及效率等各方面综合情况,其值为
EROI=∑ni=1RiVi∑ni=1IiVi, (1) 式中:Ri为光伏电站第i项系统产出当量;Ii为光伏电站第i项系统投入当量;Vi为第i项产出当量或投入当量的太阳能值转换率,sej/单位.
1.2 能量系统边界及影响因素
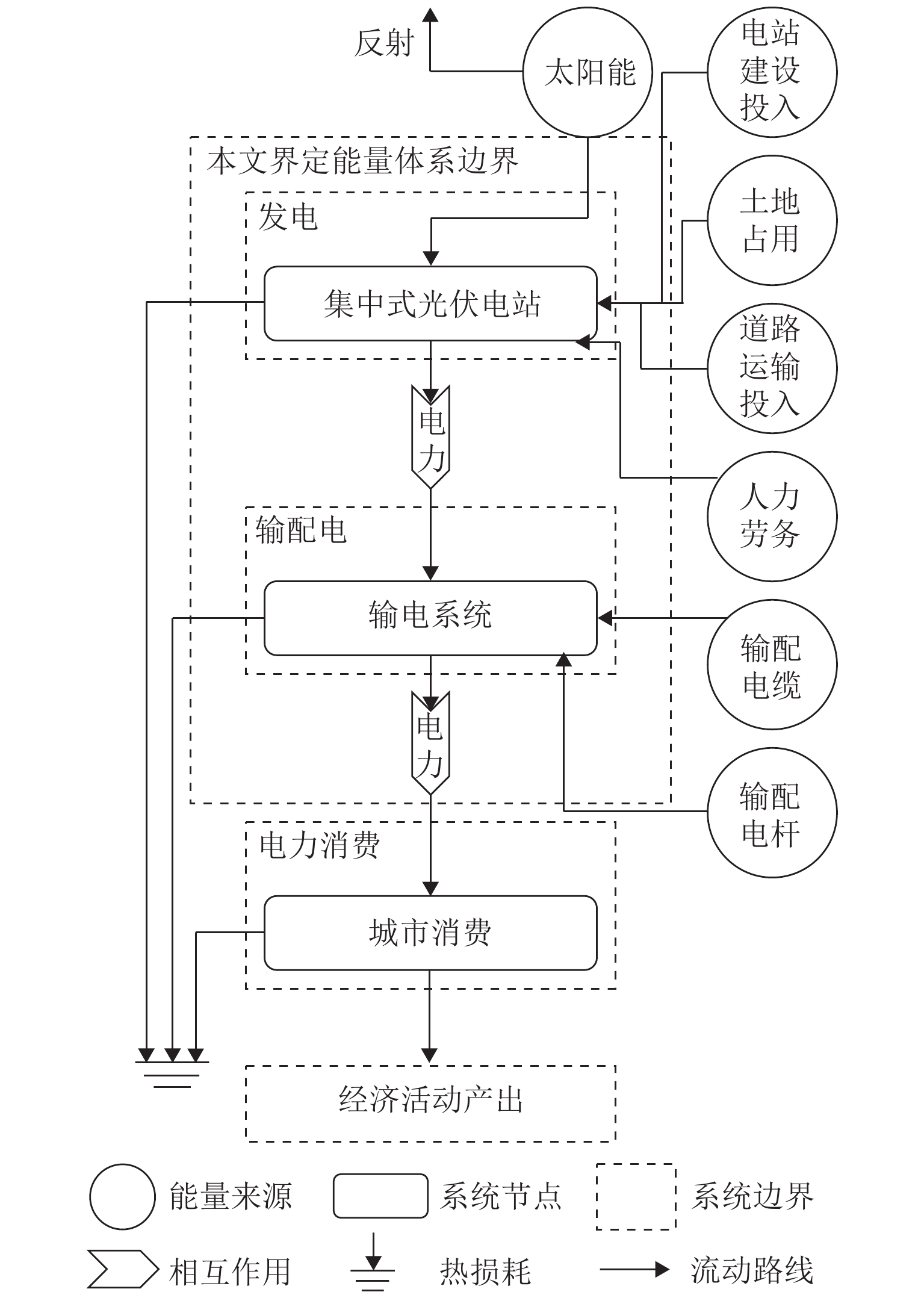
光伏电站系统的运行是一个相对复杂的过程. 通常情况,技术潜力是衡量某项特定技术实际能源回报的常用指标,即仅考虑地理、土地利用性质及系统性能等条件限制,光伏电站在技术理论上可产出的最大电力[14]. 但对于光伏发电系统而言,技术潜力仅局限于电力产出这一单一环节,明显地忽略了如组件生产、电站装机等上游系统的能值投入. 因此,本文对技术潜力进行扩展,将光伏发电能量系统边界拟定为电站建设—电站运行—电力输配—城市终端电力获取的全过程,以城市消费终端实际获取电力作为该系统的唯一产出,并认为光伏发电与燃煤、燃油等火力发电技术的度电产出,对于人类社会经济活动具有相同的贡献价值,以火电能值转换率对城市终端电力获取进行折算(图1).
此外,光伏电站生态经济收益影响因素众多,为简化评估过程,本文提取了既有研究中的主要影响因素,并将其划分为电站建设、新增道路建设及运输、新增输电线路建设及线损、电站使用期间土地占用4个主要方面,分别对其能值投入作出评估.
1.3 影响因素量化
本文以我国内蒙古地区为研究对象. 为便于评估,将电站光伏组件统一拟定为目前性价比较高的多晶硅光伏板,组件型号为JKM270PP-60-DV,组件尺寸1646 mm × 992 mm × 8 mm,峰值功率270 W,光电转换效率16.54%[15]. 研究数据依据本文拟定光伏电站系统边界进行选取,主要包括地表覆盖栅格数据[16],倾斜表面年总辐射栅格数据[17],生态功能保护区与国家级自然生态保护区矢量边界[18-19]、内蒙古地区DEM (digital elevation model)数字高程数据及道路网络、变电站、建成区分布等相关基础设施信息. 此外,各类影响因素能值投入量化方法及其能值转换率涉及电力、生态等多项领域,其计算取值参考来自相关文献研究及设计规范[20-35],能值投入产出量化方法见表1,能值转换率取值见表2. 表1中:Ve为电力能值转换率,sej/(kW·h);Api为像元面积,300 m× 300 m;L为光伏组件长度,取值3292 mm;Cp为光伏组件峰值功率,取值270 W;Ap为每光伏组件面积,1646 mm × 992 mm;Gti为倾斜表面年日照辐射总量,kW·h/(m2·年);j为光电转换效率,取值16.54%;K为光伏系统综合效率,取值75%;CL为某区域光伏电站建设的劳动力资本投入,详见第1.3.3节;VL为货币的能值转换率,sej/€;Dr为某区域光伏电站与其最近既有道路的距离,km;Vrc为道路建设的能值转换率,sej/km;Fv为单车组件运输量,取值380 块/车[15];Qs为4 × 2半挂牵引车综合油耗标准,取值0.359 L/km[36];ρf为柴油密度,取值0.8425 kg/L;Vf为柴油能值转换率,sej/kg;Jec为导线经济电力密度,取值1.2 A/mm2;T为光伏电站所处区域的年日照时数[28],h; ϕ为电流与电压之间的相位差,cos ϕ为评价电网功率因数,取值0.9;U为新增输电线路的线电压,kV;r为铜导线电阻率,取值17.24 Ω·mm2/km[26];Dt为某区域光伏电站与其最近变电站的距离,km;η0为变压器评价功率损失率,取值6%[27];ρcu为铜导线密度,取值8.93 g/cm3;Vca为电缆能值转换率,sej/kg;Mpo为单位公里电杆用钢量,取值1 560 kg/km[25];Vpo为钢的能值转换率,sej/kg;ηn为国家电网综合网损率,本文为蒙东电网5.50%、蒙西电网3.93%[29];Vic为某类地表植被年均固碳量,tC/m2;Vc为固碳量能值转换率,sej/tC.
表 1 本文估量化方法Table 1. Methods for evaluation used in this study量化类项 量化公式或取值方法 年电力能值产出/(sej·年−1) Et=EVe 光伏阵列间距/mm D,文献[36] 光伏安装面积/m2 S=ApiL/D 光伏装机容量/W C=CpS/Ap 年光伏发电量/(kW·h) E=SGtijK 电站第 i 项物质投入/(g·m−2) Mi,据文献[20]折算 第 i 项物质能值转换率/
(sej·g−1)Vi 电站建设能值投入/
(sej·年−1)Es=S(1.02∑ni=1MiVi+CLVL) 道路建设能值投入/sej Erc=DrVrc 运输能值投入/sej Ert=2Dr[C/(CPFv)]QsρfVf 道路及运输能值投入/sej Er=Erc+Ert 导线截面面积/mm2 a=I/Jec 电站年均有功功率/(kW·年−1) P=E/T 输电线路线电流/A I=P/(√3Ucosϕ) 导线电阻/Ω R=rDt/a 输电线路热损失/kW Q=3I2R 升压变电热损失/kW P0=η0(P−Q)/cosϕ 输电线路能值投入/sej Egc=Dt(ρcuaVca+MpoVpo) 年输电损失能值折算/
(sej·年−1)Eq=E[(Q+P0)/P+ηn]Ve 土地占用能值投入/sej El=ApiLciVc 年固碳量/(tC·m−2·年−1) Lci,依据文献[22-23]折算 表 2 本文能值转换率取值Table 2. Unit emergy values used in this study能值类项 能值转换率 来源 火力发电/(sej·(kW·h)−1) 1.03×1012 文献[20] 铝/(sej·g−1) 2.15×1010 文献[30] 玻璃/(sej·g−1) 3.20×109 文献[31] 硅/(sej·g−1) 1.39×1010 文献[20] 铜/(sej·g−1) 2.30×1011 文献[30] 粉尘/(sej·g−1) 1.23×1011 文献[20] 钢/(sej·g−1) 2.12×109 文献[30] 石灰石/(sej·g−1) 5.26×108 文献[32] 废气/(sej·g−1) 1.15×1014 文献[12] 盐酸/(sej·g−1) 2.14×108 文献[33] 货币/(sej·€−1) 1.02×1012 文献[34] 道路建设/(sej·km−1) 2.92×1018 文献[25] 柴油/(sej·kg−1) 3.52×1012 文献[32] 电缆(铜)/(sej·kg−1) 6.79×1010 文献[25] 电杆(钢)/(sej·kg−1) 6.72×1012 文献[25] 固碳/(sej·tC−1) 4.96×1014 文献[35] 1.3.1 可利用地选择
出于生态功能、生态物种及自然遗迹等方面保护需要,当对生态功能保护区和自然生态保护区内光伏电站的装机建设进行严格限制. 依据《光伏发电站设计规范》(GB 50797—2012)[37],光伏发电可利用空间区域主要包括河流及湖泊水面、沿海及内陆滩涂、盐碱地、沼泽地、沙地及裸地等未利用土地类型. 但结合区域气候特征、电站建设现状与本文田野调查,内蒙古地区存在大量盐碱化或植被覆盖稀疏的耕地、草原等土地类型被用于光伏电站装机建设,其用地性质仍属未利用地,而类似情况也存在于文献[38-39]中. 同时,出于生态功能、生态物种及自然遗迹等方面保护需要,还当对生态功能保护区和自然生态保护区内光伏电站的装机建设进行严格限制. 因此,考虑实际因素,本文将光伏电站可利用区域拟定为除生态功能保护区、国家级自然生态保护区与城市建成区以外的全部荒漠、裸地(苔原)、稀疏耕地及稀疏草原,并以全球地表覆盖数据对其进行粗略表征. 此外,考虑过于陡峭的地形可能增加电站施工建设与维护方面的操作难度,依据文献[40]取值,将对平均坡度大于等于25° 的地块区域予以排除.
1.3.2 电力能值产出
集中式光伏电站利用自然界未利用土地进行装机建设,其阵列排布方式受地理条件影响较大. 为模拟不同坡度、坡向地形条件下光伏电站装机建设布局,本文参考文献[36]方法,基于每处可利用地像元平均坡度及坡向提取结果,对其光伏阵列间距进行计算. 其中,组件串联方式统一拟定为目前较为常见的双排串联方式,即光伏阵列倾斜面长度L均为2倍组件长度(L=3292 mm). 而光伏组件最佳倾角参照文献[37]推荐方法,按每处像元所处纬度减去3° 进行计算. 之后,依据表1进一步对其光伏组件安装面积、装机容量、年发电量等相关参数做出评估,结合火电能值转换率对其年电力能值产出量进行折算.
1.3.3 电站建设能值投入
光伏电站建设过程中所涉及的光伏组件、支架结构、连接线缆、逆变器及汇流箱等相关设备来自原材料开采、组件加工等上游产业的物质能量投入,作为生态系统整体可持续性评价,应将其视作光伏发电系统的固有成本纳入计算. 依据文献[20]研究进行折算,本文拟用的光伏组件单位安装面积(1 m2)第i项物质投入(Mi)包含支撑材料(铝)25000.00 g、框架材料(铝)3173.11 g、玻璃6943.31 g、硅835.07 g、铜30.88 g、粉尘46.66 g、非铁金属(铝)14.16 g、钢0.02 g、石灰石等惰性物质32.50 g、废气20.00 g及盐酸等必要化学物质3500.00 g. 同时,目前我国光伏组件安装的劳务费用约0.7~0.9 元/m2,本文以0.85 元/m2(0.11 €/m2)的平均值进行计算. 此外,逆变及布线等其他设备相对投入较少,参考文献[21]取值,按总物质能值投入的2%对其进行大致评估.
1.3.4 道路及运输能值投入
出于光伏电站可达性与建设需求,通常需要依据既有道路网络新建公路以满足物质运输及建设需要. 利用ArcGIS成本路径分配功能,以DEM数据为表面栅格,可实现各地块像元与既有道路网络地理间距的提取,结合相关能值转换率,可计算其新建公路能值投入;而就运输部分能值投入评估,则依据各像元可实现的光伏装机组件数量,参考本文拟用光伏组件单车组件运输量、车辆综合油耗标准等相关运输要求,按地理距离提取结果进行计算.
1.3.5 输电及线损能值投入
集中式光伏发电需要依赖电力网络进行调度,需新增输电线路,将电力产出输送至附近升压变电站进入输电系统,并传递至城市负荷终端. 为简化计算,本文将新增输电线路统一拟定为应用较为广泛的三相四线制10 kV铜导线输电线路. 其中,新增输电线路建设主要计入电缆与电杆两部分能值投入,按电站输出功率不同,其导线截面面积相应有所差异;输电线损部分则主要计入新增输电线路段输电损失与进入国家输电系统后变压、输电损失两部分. 前者可依据各像元光伏电站的年平均输出功率计算得出,而后者则依据变压器平均功率损失、像元所处地区综合网损率等参数粗略评估.
1.3.6 土地占用能值投入
除上述影响因素外,光伏电站的运行周期较长、占用土地面积通常较大. 由此所导致的生物多样性缺失、地表植被及土壤层破坏等次生影响同样需要被视作一项重要的生态经济投入纳入考虑. 但因其涉及种类繁多、尚难以量化,故本文仅将其土地占用期间因地表植被移除所产生的年固碳减少量这一生态收益损失计入土地占用能值投入. 其中,各类地表植被单位覆盖面积(1 m2)年固碳量参考文献[22],由全球森林年均固碳量[23]推算得出.
1.4 生态经济收益评估
基于各分项能值指标量化结果,按25年光伏组件使用周期,对式(1)进行扩展. 其中,电站建设投入、道路及运输、新增输电线建设为电站建设初期的一次性投入;而光伏电力产出与输电损失则存在于全部生命周期当中;考虑其原有地表覆盖将可能长期处于无法修复状态,将土地占用的时间维度扩展至100年,并依据生态足迹方法相关要求[23],将额外12%的生物多样性保护面积损失纳入考虑范畴. 各地块像元光伏电站能值产投比可由式(2)计算得出.
EROI=25EtEs+Er+Egc+25Eq+112EL, (2) 式中:EL为土地占用年固碳量损失能值折算量,sej/年.
2. 评估结果
内蒙古地区光伏电站可利用面积为605665 km2,约占其总面积的52.80%. 但考虑电站实际生态经济收益(EROI>1),其中具备开发价值的土地面积为 223646 km2,约占总面积的19.50%. 为进一步模拟未来各盟市光伏电站开发情况,本文以我国2050年光伏发电量占比39%的总体发展目标为依据,并结合不同地区用电负荷增长预测,对各盟市光伏电站可利用地面积、可开发面积及2050年光伏电站开发面积等作出综合描述(表3). 表中:2050年全社会用电量以电力消费弹性系数法估算得出,以2019年各盟市全社会用电量为基期数据参考,该数据来自内蒙古自治区能源局[41];年均GDP增长率取自各盟市2014年—2018年平均水平;2050年光伏电站用地面积以各盟市分区可开发土地EROI值降序排列,按年发电量预测值累加至其2050年全社会用电量的39%进行评估. 值得说明的是,考虑光伏电力将主要输配至与其最近的盟市中心城区,因此,表3预测结果并非以行政分区作为城市划分依据,而是基于ArcGIS成本路径分配功能,以各电站(像元)所处区域与其相邻盟市中心距离决定该电站的分区归属.
表 3 各盟市光伏用地概况及2050年发展预测Table 3. PV land survey and 2050 prospect of each league city盟市 土地面积统计/km2 2050 年用电量预测/
(×109 kW·h)2050 年光伏用地面积/km2 分区
面积可开发
土地呼和浩特 55797 15929 3318.1 593.4 包头 45061 28101 10451.0 1872.2 呼伦贝尔 236431 17741 880.2 206.5 兴安盟 97904 20164 598.3 125.3 通辽 62749 7784 3478.7 729.9 赤峰 58250 5061 2218.0 449.4 锡林郭勒 170328 37332 1800.4 353.2 乌兰察布 51026 14189 6441.6 1247.6 鄂尔多斯 37530 16601 9625.4 1802.6 巴彦淖尔 83092 29458 1658.2 238.2 乌海 48620 17411 4933.4 987.4 阿拉善 199674 13874 1833.9 321.0 总计 1146462 223645 47237.2 8926.7 由表3可知:可开发土地面积占比最高的为内蒙古中西部地区;而西部阿拉善地区由于城市密度低、电站开发成本投入较大,其可开发土地占比较低;呼伦贝尔、通辽、赤峰、锡林郭勒等东北部及东部地区由于保护区限制及可利用土地较少,其整体可开发土地占比也处于较低的水平. 此外,以我国2050年光伏发展目标来看,光伏用地开发面积占比呈现出不同程度的差异;如鄂尔多斯、包头两处光伏用地开发面积占比分别需达到各自分区面积的4.80%及4.15%,方可满足光伏电力占比39%的发展预期;而阿拉善、兴安盟及呼伦贝尔等地仅需0.10%左右. 原因在于各地区光伏可利用土地面积的不同,而更为主要的原因则在于地区经济发展速度不同所导致的用电负荷需求差异. 就内蒙古地区总体范围而言,开发约0.78%的土地面积即可满足自身2050年光伏发展目标. 但对于我国经济发展速度更快、可利用地更为紧缺的东部地区而言,仅凭本地区光伏电站开发可能难以满足,而为实现全国范围2050年总体光伏发展目标,内蒙古地区实际所承担的光伏电站开发量将更为巨大. 有效利用EROI能值分析方法进行选址评估,可在实现其电力发展目标的同时,最大程度保障光伏发电生态经济收益,对于优化光伏能源的整体空间部署具有重要的指导意义.
3. 讨 论
3.1 与既有评估方法对比
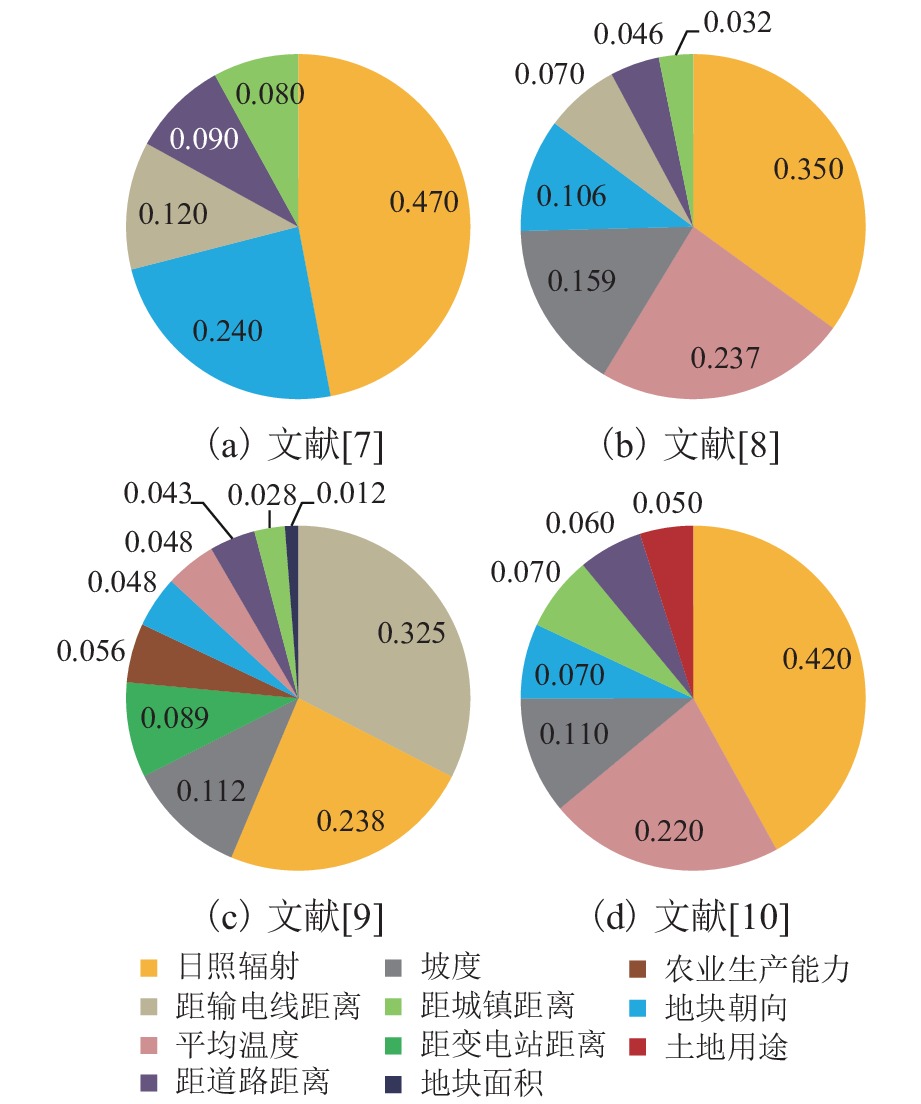
既有MCDM由于各项决策性指标(即影响因素,如日照辐射强度、距输电线距离、距道路距离等)并非处于同一度量维度,存在能量类别与价值的根本差异,无法简单直接加减. 因此,在具体评估时,需要将各项决策性指标乘以一个相对应的权重,以反映不同决策性指标对于最终综合评价值的影响程度,如式(3)所示. 其中,权重的制定以AHP应用最为常见. 该方法依据参与评估的决策性指标类别,对复杂问题建立主观判断结构,之后依据专家(或从业者)的比较结果,建立判断矩阵,并通过一致性检验,最终依据几何平均法、特征向量法及算数平均法等确定各项决策性指标所占权重向量. 因此,权重值的大小对最终评估结果的影响至关重要.
S=∑Nn=1CnWn, (3) 式中:S为某电站选址地块的综合收益评价值,Cn为某电站第n项决策性指标的评估值,Wn为第n项指标所占权重值.
权重值的确立因参与评估者认知背景及劳务关系(政府、开发商或研究人员)等各方面差异会产生不同的变化. 图2为不同研究中关于光伏电站选址各项决策指标权重取值的对比. 从图2可以看出:除文献[9]外,日照辐射条件均占据最高权重,而其他决策性指标的权重取值和排序却呈现出不同程度的变化. 尽管一些研究人员采用了其他方法加以改善,如消去与选择转换法(ELECTRE)[42]、有序加权平均法(OWA)[43]及优劣解距离法(TOPSIS)[44]等,但其效果并不显著,原因在于权重标准是MCDM的基础,而人们已经习惯以市场经济价值衡量环境资源所禀赋的生态经济价值,加之个人主观因素的影响,其评估结果难以具备可信度与科学性. 此外,MCDM评判分值的本质只是一个相对的比较值,其本身并不具备任何表征意义,也不能用来回答任何实际生态经济收益状况、实际收益量为多少等. 而相比之下,EROI分析法通过模拟及对比光伏电力系统运作过程中的各项能值投入与产出,并以太阳焦耳作为标准,将生态环境系统与人类社会经济系统有机统一,为衡量和表达光伏电力系统生态经济收益提供了共同的度量标准.
3.2 度电能值分析
表4为本文抽取的内蒙古地区不同EROI水平下光伏度电能值及其构成情况样本数据. 光伏度电能值越大,代表同等电力产出情况下的生态经济代价投入与环境负载越大,越不宜优先开发. 由此可以量化分析城市终端每度光伏电力来源的能值投入构成情况,并同其他技术形式的同等电力产出进行对比,为技术类型选择、光伏电站区位部署及其能源空间规划提供更为直观科学的指导依据.
表 4 光伏度电能值构成及同火力发电对比Table 4. Emergy composition per unit PV power generation and comparison with thermal powerEROI 度电能值构成/(sej·(kW·h)−1) 光伏度电能值/
(sej·(kW·h)−1)占同等火力
发电比例/%电站建设 土地占用 道路/运输 新增输电线路 输电线损 1.00 6.40×1011 2.14×109 3.84×1011 2.94×109 2.21×1011 1.25×1012 100.0 1.00 6.76×1011 1.75×109 3.49×1011 3.43×109 2.68×1011 1.30×1012 100.0 1.20 6.63×1011 6.55×108 1.57×1011 1.12×109 2.44×1011 1.07×1012 79.8 1.20 6.89×1011 8.05×108 1.13×1011 2.39×109 3.52×1011 1.16×1012 78.1 1.40 6.49×1011 9.12×108 1.82×1010 1.54×109 2.44×1011 9.13×1011 65.0 1.40 6.17×1011 5.87×108 4.69×1010 1.10×109 2.56×1011 9.22×1011 64.7 1.60 5.68×1011 4.78×108 1.91×1010 2.51×108 1.57×1011 7.44×1011 57.0 1.60 5.66×1011 5.22×108 2.08×1010 2.73×108 1.57×1011 7.44×1011 57.0 1.73 5.42×1011 1.50×108 0 3.75×107 1.27×1011 6.69×1011 52.6 平均值 6.41×1011 7.06×108 6.00×1010 1.48×109 2.60×1011 9.63×1011 68.3 注:数据为基于本文评估结果的抽样数据示意,平均值统计包含全部EROI大于1.00地块. 由表4可知,光伏电站的度电能值与其选址密切相关. 依据度电能值构成的平均值结果,在光伏度电能值的各项构成中,电站建设的能值投入占据主导地位. 这符合能值分析的理论基础,即能量等级. 基于热力学第二定律,在能量转换链中伴随能量的传递与转换,其能量等级逐渐提高,而能量数量逐渐减少. 因此,能量系统中的高等级者具有更大能值转换率,需要更多的能量投入得以维系. 光伏组件、逆变器等发电设备经过一系列上游人类社会工业生产,涉及大量人类劳动、科技信息及其他高级技术设备,处于光伏发电能量系统中较高的功能地位及能量等级. 但这项投入通常仅与地形条件相关,如表4抽样结果所示,电站建设作为一项固定成本投入在不同EROI水平下并未体现出明显的差异. 除此之外,光伏电站的生态经济收益更大程度取决于输电线路距离及由此所产生的输电损失. 其原因在于,依据本文拟定条件,城市消费终端实际获取电力是光伏发电能量系统的唯一产出,扮演着系统中最为核心的功能地位. 尽管依据本文评估结果,内蒙古地区的平均输电损失仅约每公里0.4%,但作为最高等级的系统能量损失,其重要程度足以影响光伏电站生态经济收益与区位选择. 另外,由于本文仅将年固碳量损失计入土地占用成本投入,其度电能值构成占比较低. 但事实上,因光伏电站装机建设所导致的生态环境改变、物种多样性缺失、人文生态景观破坏等问题可能是永久性的,其实际影响或将更为严重,因此,在具体电站选址中仍需结合其地理生态资源环境因地制宜,做出妥善评估. 通过能值构成分析可以发现,各类因素对于光伏电站选址收益的影响程度事实上并非如同MCDM那样,将决策指标与权重进行乘积后所呈现出的线性增长;其内在本质是对不同影响要素所处能量等级与其所包含具体能质的量化评估,而以生态价值作为评判标准,具体因素对选址评估所产生的影响差异或将远大于权重指标.
3.3 对能源空间规划的启示
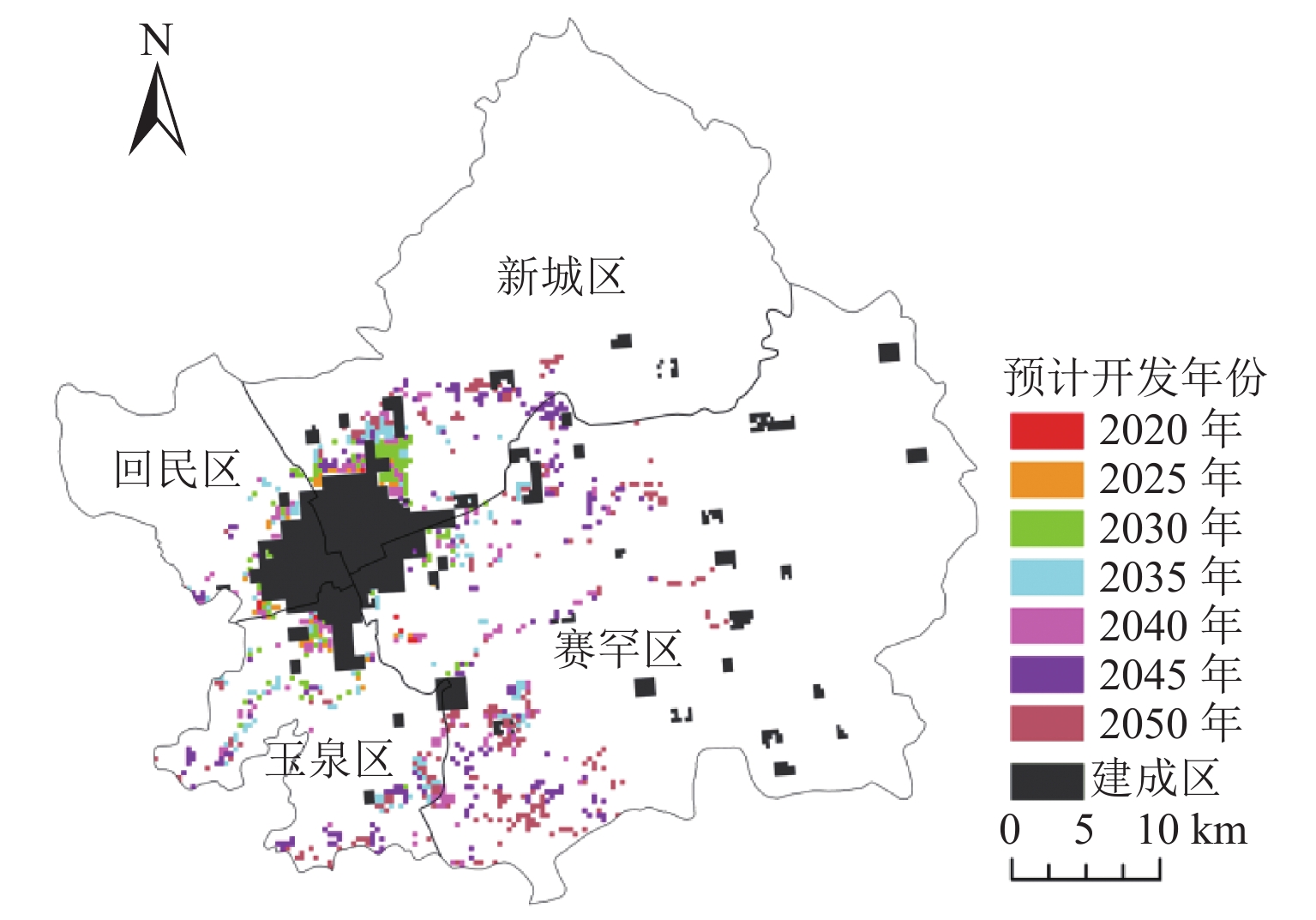
光伏电站生态经济收益度的量化评估是指导其有序规划的必要基础. 本文以火力发电作为对比参照,当EROI趋近于1.00时,光伏电站生态经济收益与火力发电近乎相同,并未体现出明显的优势性. 而伴随EROI水平升高,光伏电站生态经济收益才得以逐步提升. 如前文论及,输电损失是影响光伏电站生态经济收益最为重要的因素,其次为道路及运输影响. 而通常情况下,受电力调配需要影响,变电站等电力接入基础设施会就近布置于城镇或建成环境附近区域,以降低输电损耗. 而距城镇越近的区域,其路网分布也将更为密集. 由此,光伏电站选址区域距城镇距离将成为影响光伏能源空间布局的重要因素. 呼和浩特市周边地区光伏用地逐年发展规划预测情况如图3所示.
从图3可以看出:与城镇较近的区域选址收益相对更高,而具备更高的开发优先级. 但事实上,电力需求与城镇化进程具有一定程度的内在关联. 城镇化进程的加剧会导致电力需求的增加,出于能源可持续的发展需要,对于光伏能源的建设需求也将更加迫切. 基于这种考虑,不断扩张的城市建设与光伏电站间的用地冲突,可能进一步成为未来光伏与城市空间规划共同面临的首要问题. 保障光伏用地土地性质的同时,兼顾其周边发电环境免受城镇建设的影响,或将成为未来空间规划者们新的研究重点.
4. 结 论
本文将能值理论与光伏电站生态经济收益评估相结合,可为未来光伏电站选址、政策指标制定及光伏空间规划等方面提供更为科学有效的理论指导与决策支持. 并通过具体案例分析指出其研究思路的可行性.
在影响因素与能量系统边界的拟定上仍存在诸多局限. 本文仅以火力发电为标准,作为衡量光伏电站选址生态经济收益的参照标准,而在实际应用过程中,尚需兼顾如水电、风电等其他发电技术形式作为对比参照,整合更多影响因素并扩展其能量系统评估边界. 此外,还应考虑农光、渔光互补、光伏与固沙治理等复合型电站中其他生态产品产出、生态危害减少等方面所带来的积极生态收益,视具体情况对单位土地面积能值产投比作出更为严密的分析.
可以初步认为这种评估思路具备可行性. 通过评判标准与价值标准的转变,EROI能值分析可进一步为未来光伏能源的空间部署带来新的规划认知理念,并将在未来科学指导能源结构转型、推进城镇化与生态环境建设和谐发展过程中发挥积极作用.
-
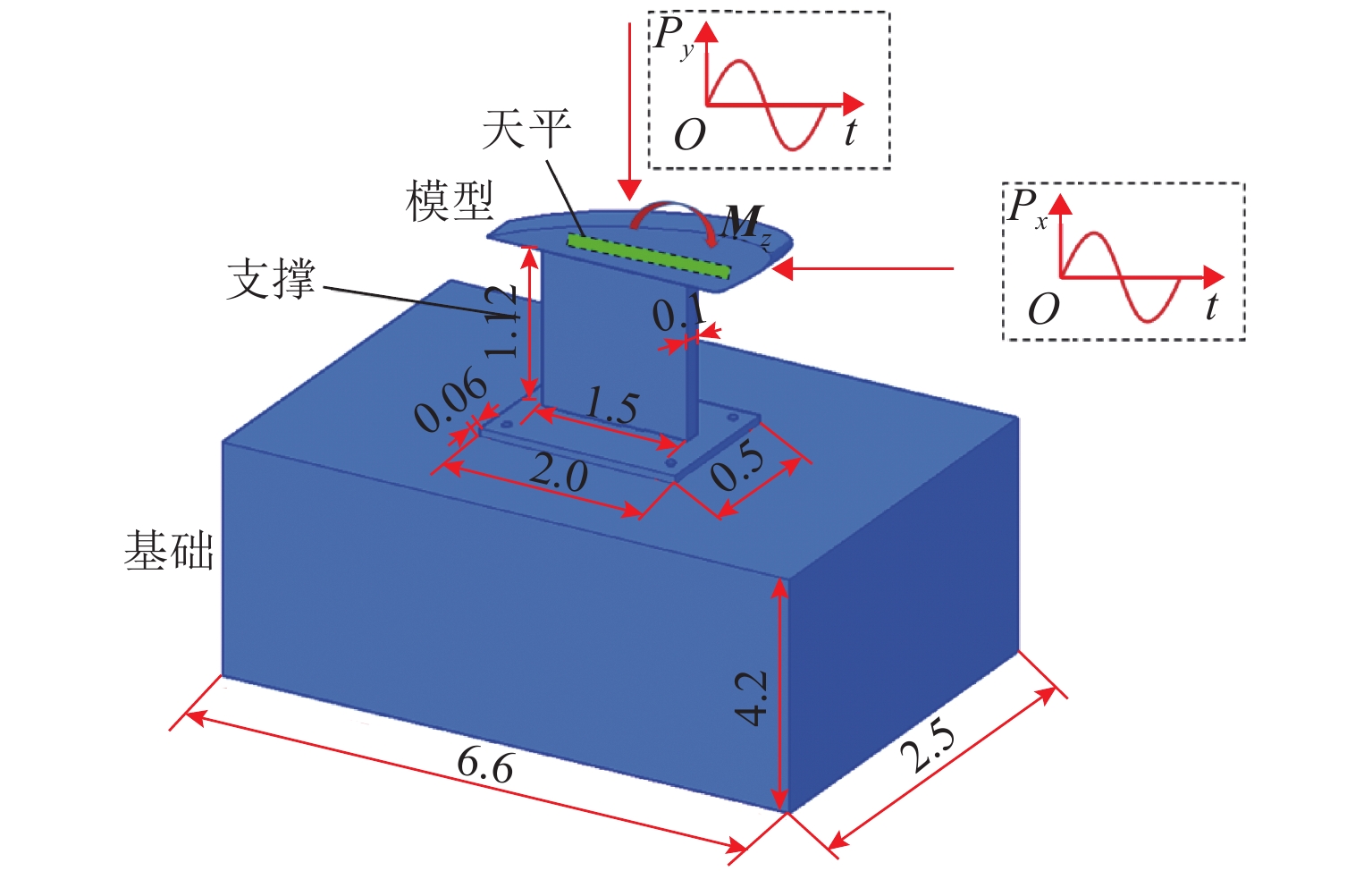
表 1 网格无关性检验方案及计算结果
Table 1. Test scheme and calculation results of mesh independence
方案 节点
数/个单元
数/个竖直向最大振幅/μm 水平向最大振幅/μm 回转角度最大振幅/(°) 1 12749 17392 1.71 2.78 3.1×10−4 2 18308 24878 1.75 2.83 3.4×10−4 3 32637 44583 1.77 2.86 3.6×10−4 4 59842 78949 1.78 2.87 3.6×10−4 表 2 材料计算参数
Table 2. Parameters of materials
名称 重度/
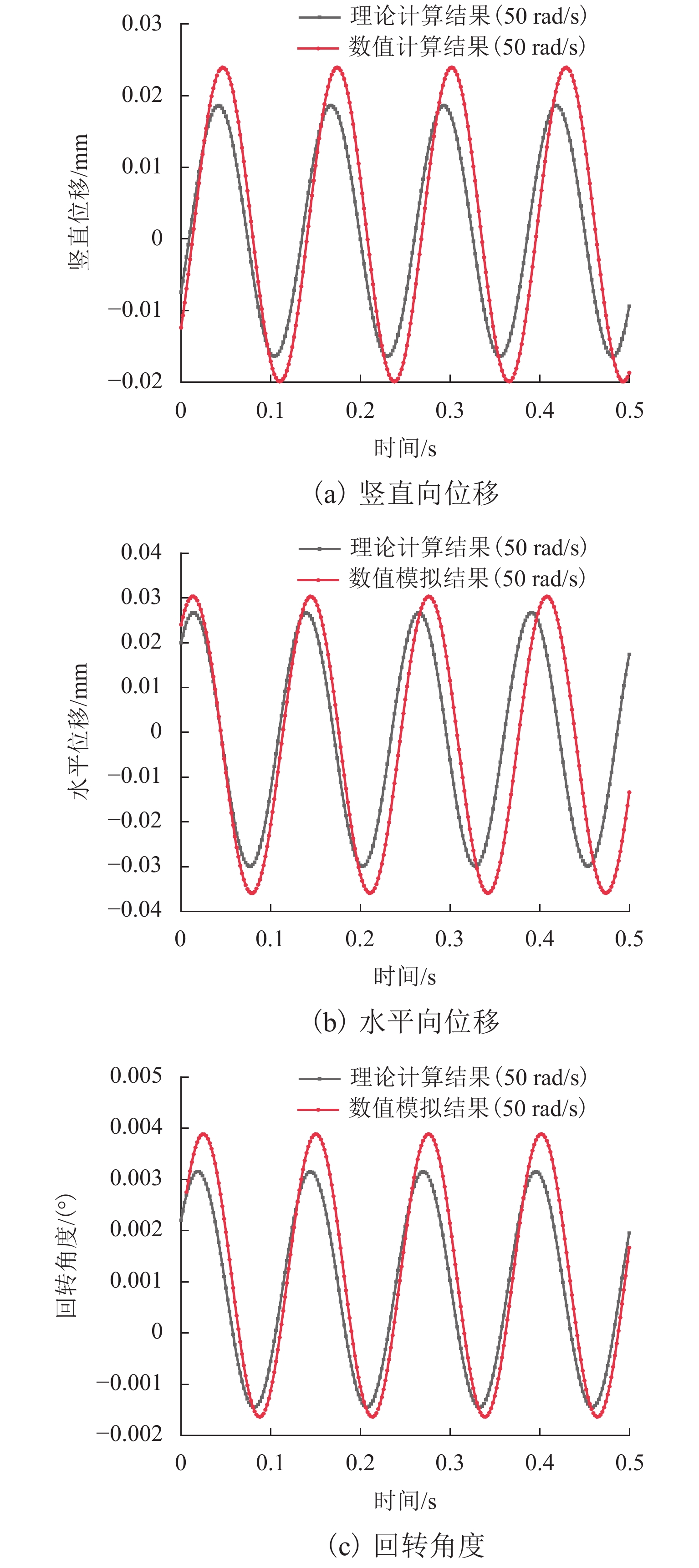
(kN·m−3)弹性模量/GPa 泊松比 剪胀角/(°) 偏心率 fb0/fc0 K 黏性系数 支撑 78 210 0.2 地基 19 30 0.25 38 0.1 1.16 0.67 0.005 基础 25 30 0.25 38 0.1 1.16 0.67 0.005 表 3 理论计算结果与数值模拟结果对比
Table 3. Comparison of theoretically calculated results and numerically simulated results
方法 频率/Hz 最大振幅/μm 竖直向 水平向 回转角 竖直向 水平向 回转角 理论计算 8.06 1.75 7.94 0.00283 7.94 3.4×10−4 数值模拟 7.81 2.19 7.75 0.00331 7.94 4.1×10−4 表 4 不同基础尺寸计算结果
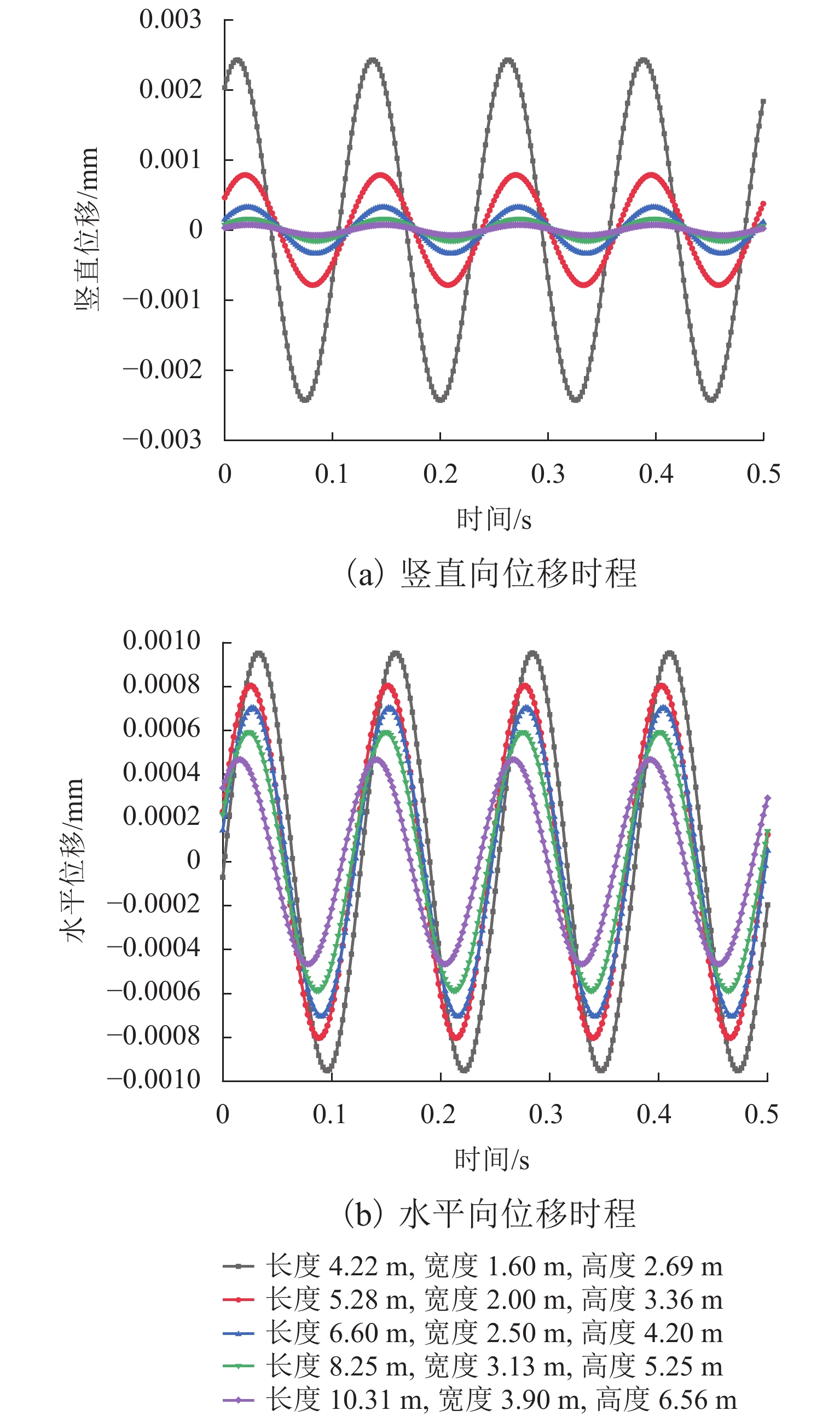
Table 4. Calculation results of foundation with different sizes
基础尺寸 最大振幅/μm 频率/Hz 竖直振动 水平振动 竖直振动 水平振动 0.64S 2.24 6.45 9.52442 7.48 0.80S 0.787 5.35 8.03606 6.21 S 0.331 4.49 7.03641 5.22 1.25S 0.155 4.02 5.89714 4.66 1.56S 0.075 3.59 4.66436 4.17 -
[1] 乐嘉陵. 吸气式高超声速技术研究进展[J]. 推进技术,2010,31(6): 641-649.LE Jialing. Progress in air-breathing hypersonic technology[J]. Journal of Propulsion Technology, 2010, 31(6): 641-649. [2] GAZETAS G. Analysis of machine foundation vibrations: state of the art[J]. International Journal of Soil Dynamics and Earthquake Engineering, 1983, 2(1): 2-42. doi: 10.1016/0261-7277(83)90025-6 [3] MIZUNO H. Effects of structurefh soil-structure interaction during various excitations[C]//Proc., 7th World Conference on Earthquake Engineering. Istanbul: [s.n.], 1980: 149-156. [4] NII Y. Experimental half-space dynamic stiffness[J]. Journal of Geotechnical Engineering, 1987, 113(11): 1359-1373. doi: 10.1061/(ASCE)0733-9410(1987)113:11(1359) [5] SARRAZIN M A, ROESSET J M, WHITMAN R V. Dynamic soil-structure interaction[J]. Journal of the Structural Division, 1972, 98(7): 1525-1544. doi: 10.1061/JSDEAG.0003278 [6] VELETSOS A S, TANG Y. Vertical vibration of ring foundations[J]. Earthquake Engineering & Structural Dynamics, 1987, 15(1): 1-21. [7] MITA A, LUCO J E. Impedance functions and input motions for embedded square foundations[J]. Journal of Geotechnical Engineering, 1989, 115(4): 491-503. doi: 10.1061/(ASCE)0733-9410(1989)115:4(491) [8] AVILÉS J, PÉREZ-ROCHA L E. A simplified procedure for torsional impedance functions of embedded foundations in a soil layer[J]. Computers and Geotechnics, 1996, 19(2): 97-115. doi: 10.1016/0266-352X(95)00038-C [9] MAUGERI M, MUSUMECI G, NOVITÀ D, et al. Shaking table test of failure of a shallow foundation subjected to an eccentric load[J]. Soil Dynamics and Earthquake Engineering, 2000, 20(5/6/7/8): 435-444. [10] GAJAN S, KUTTER B L, PHALEN J D, et al. Centrifuge modeling of load-deformation behavior of rocking shallow foundations[J]. Soil Dynamics and Earthquake Engineering, 2005, 25(7/8/9/10): 773-783. [11] BHATTACHARYA S, ADHIKARI S. Experimental validation of soil-structure interaction of offshore wind turbines[J]. Soil Dynamics and Earthquake Engineering, 2011, 31(5/6): 805-816. [12] 钱鸿缙. 动力机器基础设计[M]. 北京: 中国建筑工业出版社, 1980: 23-66. [13] 严人觉. 动力基础半空间理论概论[M]. 北京: 中国建筑工业出版社, 1981. [14] GAZETAS G. Formulas and charts for impedances of surface and embedded foundations[J]. Journal of Geotechnical Engineering, 1991, 117(9): 1363-1381. doi: 10.1061/(ASCE)0733-9410(1991)117:9(1363) [15] ÇELEBI E, FıRAT S, ÇANKAYA İ. The effectiveness of wave barriers on the dynamic stiffness coefficients of foundations using boundary element method[J]. Applied Mathematics and Computation, 2006, 180(2): 683-699. doi: 10.1016/j.amc.2006.01.008 [16] CELEBI E, FIRAT S, CANKAYA L. Dynamic impedance functions for rectangular rigid foundations[J]. Teknik Dergi/Technical Journal of Turkish Chamber of Civil Engineers, 2006, 17(2): 3827-3849. [17] 蒋东旗,谢定义. 动力机器基础设计的数值方法研究[J]. 土木工程学报,2002,35(1): 74-78.JIANG Dongqi, XIE Dingyi. Numerical simulation method for foundation design of dynamic machine[J]. China Civil Engineering Journal, 2002, 35(1): 74-78. [18] 王幼青,张克绪,朱腾明. 动力机器基础与地基体系分析[J]. 哈尔滨建筑大学学报,1999(3): 43-47.WANG Youqing, ZHANG Kexu, ZHU Tengming. Dynamic machine soil-foundation system analysis[J]. Journal of Harbin University of Civil Engineering and Architecture, 1999(3): 43-47. [19] 刘志久,尚守平,徐建. 埋置基础扭转振动的实用化计算与试验的对比[J]. 岩土力学,2011,32(12): 3618-3622.LIU Zhijiu, SHANG Shouping, XU Jian. Comparison of practical calculation for torsional vibration of embedded foundations and experiments[J]. Rock and Soil Mechanics, 2011, 32(12): 3618-3622. [20] 燕彬,黄义,王成林. 任意刚性基础竖向动阻抗的简化计算[J]. 世界地震工程,2005,21(1): 91-96.YAN Bin, HUANG Yi, WANG Chenglin. Simplified method for dynamic vertical impedances of rigid foundations with arbitrary shapes[J]. World Information on Earthquake Engineering, 2005, 21(1): 91-96. [21] 中华人民共和国住房和城乡建设部. 动力机器基础设计标准: GB 50040—2020[S]. 北京: 中国计划出版社, 2020. [22] CHEN S S, SHI J Y. A response-based simplified model for vertical vibrations of embedded foundations[J]. Soil Dynamics and Earthquake Engineering, 2011, 31(5/6): 773-784. [23] 刘庆宽,何书勇,贾娅娅,等. 防雪栅与路基间距对路基积雪分布影响规律的数值模拟研究[J]. 振动与冲击,2021,40(6): 227-234,264.LIU Qingkuan, HE Shuyong, JIA Yaya, et al. Numerical simulation on the influence of the distance between snow fence and subgrade on the snow distribution on subgrade[J]. Journal of Vibration and Shock, 2021, 40(6): 227-234,264. [24] 王毅刚,朱朗贤,王玉鹏,等. 高速列车转向架区域气动噪声源的特征识别[J]. 西南交通大学学报,2023,58(2): 261-271,286.WANG Yigang, ZHU Langxian, WANG Yupeng, et al. Characteristic identification of aerodynamic noise sources in high-speed train bogie area[J]. Journal of Southwest Jiaotong University, 2023, 58(2): 261-271,286. [25] 于梦阁,李美香,刘加利,等. 考虑风速纵、横分量的列车气动载荷变化特性[J]. 西南交通大学学报,2024,59(1): 29-35.YU Mengge, LI Meixiang, LIU Jiali, et al. Study on unsteady aerodynamic loads of high-speed trains exposed to stochastic wind for ant wind angle[J]. Journal of Southwest Jiaotong University, 2024, 59(1): 29-35. [26] 第一机械工业部设计研究总院. 动力机器基础设计手册[M]. 北京: 中国建筑工业出版社, 1983. [27] 中华人民共和国住房和城乡建设部. 建筑结构荷载规范: GB 50009—2012[S]. 北京: 中国建筑工业出版社, 2012. [28] 柯世堂,葛耀君,赵林,等. 大型冷却塔结构的等效静力风荷载[J]. 同济大学学报(自然科学版),2011,39(8): 1132-1137.KE Shitang, GE Yaojun, ZHAO Lin, et al. Equivalent static wind load of large cooling tower[J]. Journal of Tongji University (Natural Science), 2011, 39(8): 1132-1137. -






 下载:
下载:


 下载:
下载: