Dynamic Coordination Coefficient Method for Critical Buckling Load of Stiffened U-Shaped Steel Sheet Pile
-
摘要:
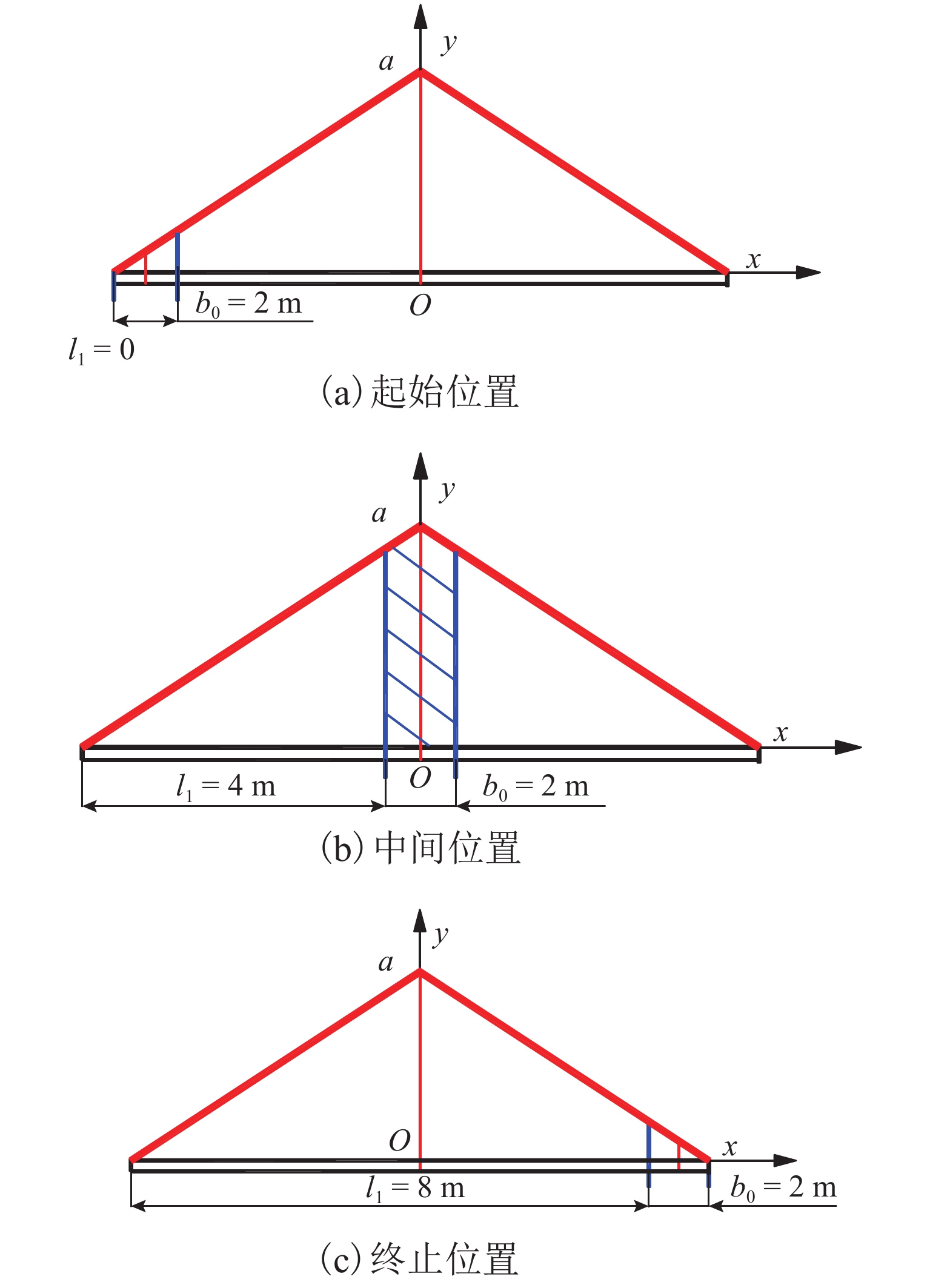
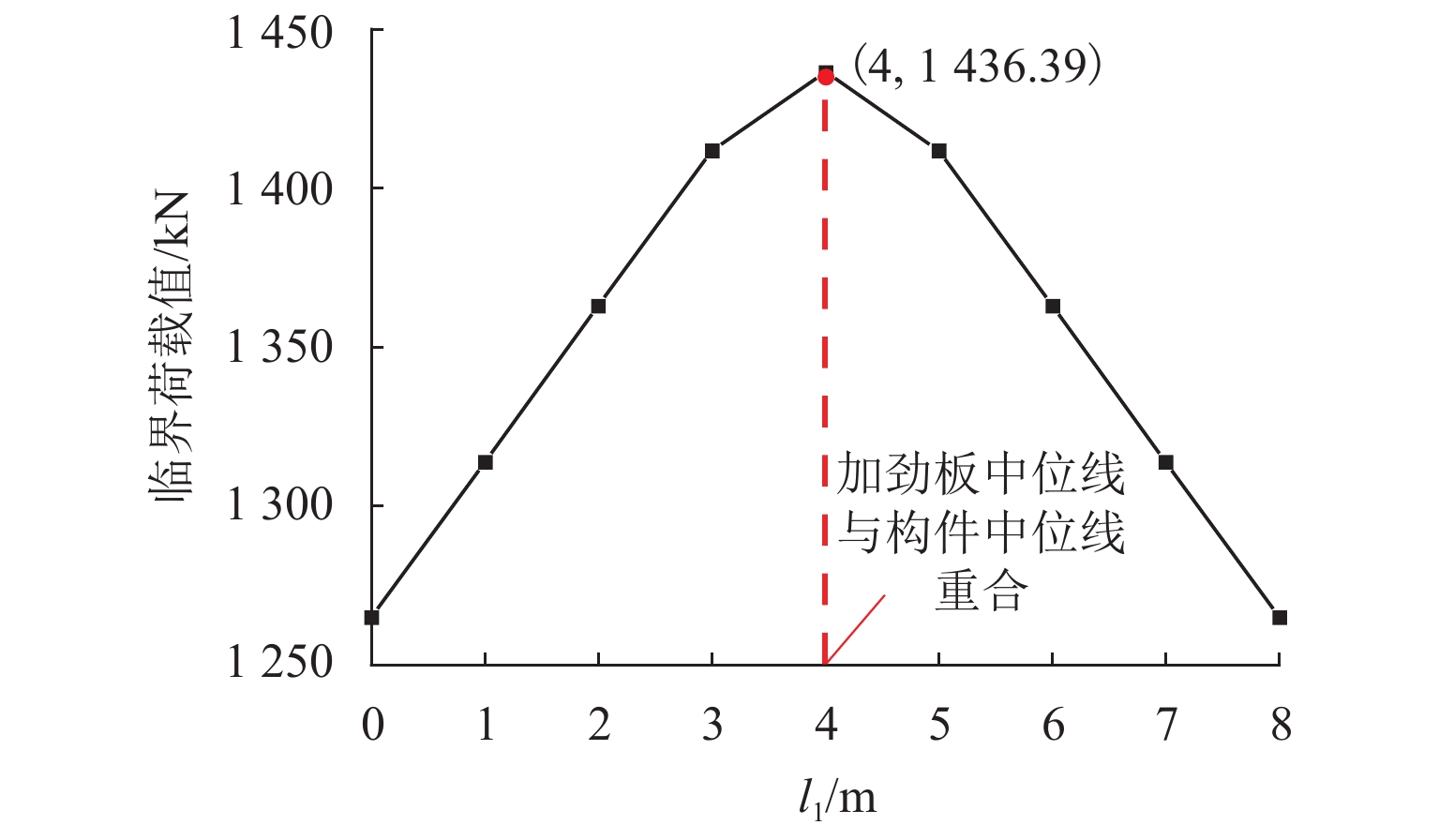
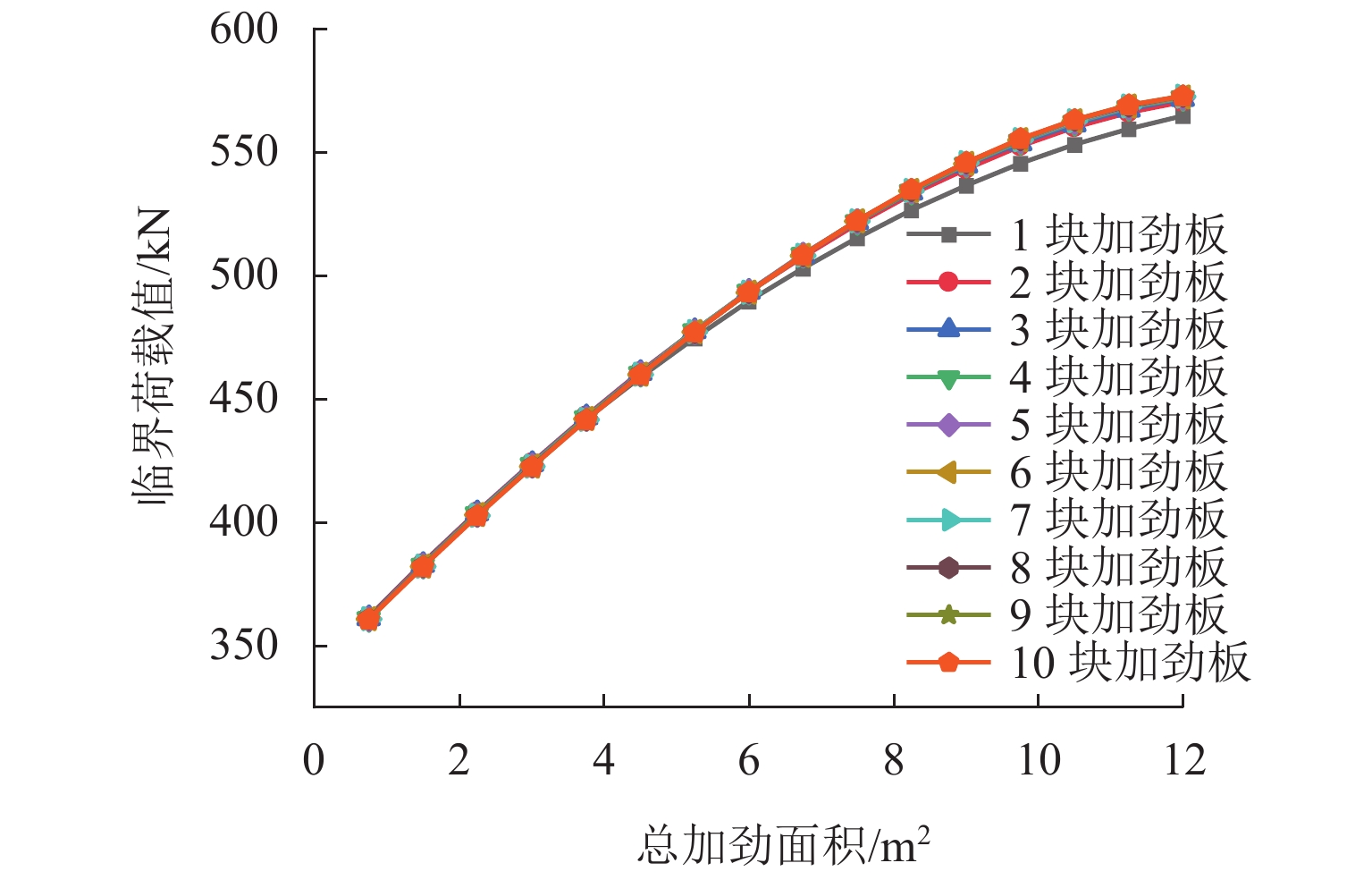
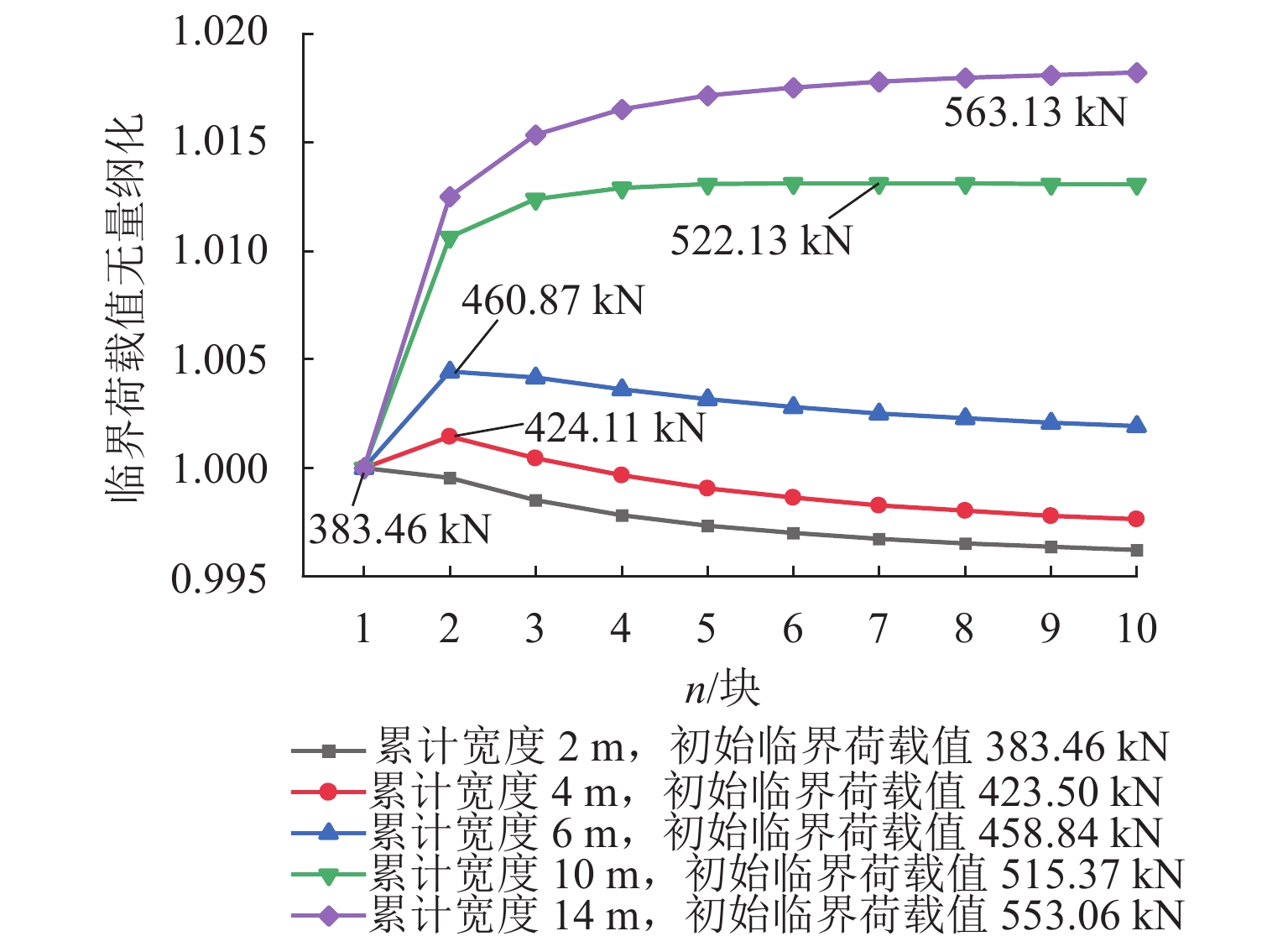
针对U型钢板桩插打容易发生屈曲的问题，提出 U型钢板桩局部加劲效应理论计算的“动态配位系数法”，建立了局部加劲U型钢板桩失稳临界荷载计算式，并分析了加劲面积、加劲位置、加劲板数量对加劲效应（即失稳临界荷载）的影响规律. 研究结果表明：加劲总面积（即加劲板累计宽度）一定时，对于长度确定的U型钢板桩，存在使构件临界荷载值最大化的加劲板布置方案，并揭示了块数太多时由于单块加劲板宽度太小而引起的局部失稳规律；以10 m长U型钢板桩为例，构件临界荷载值随单块加劲板布置位置的变化可提高13.55%，并求得20 m长的U型钢板桩不同加劲总面积对应的加劲板布置优化理论方案，为实际工程中U型板桩局部加劲方案设计提供了理论依据和参考.
Abstract:Since U-shaped steel sheet piles (USSSPs) are prone to buckle, a dynamic coordination coefficient method for theoretically calculating the local stiffening effect of USSSPs is proposed, and a formula for calculating the critical buckling load of locally stiffened USSSPs is established. The influence of different stiffening areas, stiffening positions, and number of stiffened plates on the stiffening effect (i.e., critical buckling load) is analyzed. The study shows that when the total stiffening area (i.e., cumulative width of stiffened plates) is constant, for the USSSP with determined length, there is a stiffened plate arrangement scheme to maximize the critical load value of the component, and the local buckling law caused by too small width of a single stiffened plate when the number of plates is too large is revealed. As an example, the critical load value of the component of the USSSP with a length of 10 m can be increased by 13.55% with the change of the position of the single stiffened plate, and the optimal theoretical scheme of stiffened plate arrangement corresponding to different total stiffening areas of the USSSP with a length of 20 m is obtained. This paper provides a theoretical basis and reference for the design of the local stiffening scheme of USSSPs in practical engineering.
-
目前，无砟轨道是高速铁路和城市轨道交通的主要轨道结构形式[1]. 无砟轨道作为一种多层薄板体系，层间界面为结构薄弱环节，在列车荷载和环境共同作用下，结构层间易产生离缝[2-4]. 现场调研发现，在多雨地区或排水不良地段，水容易进入轨道结构层间，形成离缝冒浆等病害，加速无砟轨道结构劣化[5-8]. 因此，研究列车荷载与水耦合作用下无砟轨道水致损伤，首要的是明确结构层间水流特性.
列车荷载作用下无砟轨道层间离缝内水流特性问题，实质上是多层薄板体系内层间裂缝内水流特性问题. 调研发现，无砟轨道层间脱空病害主要发生在板边，实际无砟轨道层间脱空区域是不规则的形状，为方便分析计算，将层间脱空区域简化为矩形等规则形状. 基于此简化模型，相关学者设计了不同层间脱空状态的混凝土试件，结合现有的加载装置开展动水压力试验. 徐桂弘等[9]设计了与原型相似的几何模型试件，离缝位于试件板边中部，离缝尺寸为300 mm × 500 mm × 3 mm （深度 × 宽度 × 开口量）. 试验过程中，施加的荷载为（60±35） kN，分析不同荷载频率下层间离缝内动水压力的变化. 发现荷载频率为15 Hz时，层间离缝动水压力最大，最大值为4.968 kPa. 该试验将混凝土层间脱空试件直接放置到水箱中，由于缺乏必要的防振动措施，试件在加载过程中会发生较为明显的振动甚至跳动，使试验测试结果与理论计算结果存在较大差异. 曹世豪等[10]在原试验装置上增设了必要的防振动措施，设计的裂缝尺寸为250 mm × 600 mm × 3 mm（深度 × 宽度 × 开口量），试验荷载为（25±10） kN，最大荷载频率为5 Hz，测得层间离缝最大动水压力为0.177 kPa，与理论计算结果较为接近. 但列车荷载具有频率高的特点，我国高速铁路设计时速在250～350 km/h，以时速250 km为例，一个转向架相邻轮载作用间隔时间为0.04 s，即荷载频率为25 Hz.
以上试验研究一方面受试验加载设备的限制，采用的荷载条件难以反映列车荷载频率高的特点，另一方面，由于设计的试件尺寸较大，在荷载作用下混凝土层间脱空区域的变形量很小，不能较好地模拟实际列车荷载作用下板边脱空区域的变形行为，也不能很好地反映实际列车荷载作用下无砟轨道层间动水压力变化. 因此，有必要基于无砟轨道层间脱空变形特性，设计能够有效模拟高频荷载作用下无砟轨道层间裂缝内水流运动的试验装置，为进一步分析列车荷载与水耦合作用下无砟轨道层间裂缝内水流特性提供试验基础.
1. 无砟轨道层间脱空力学行为
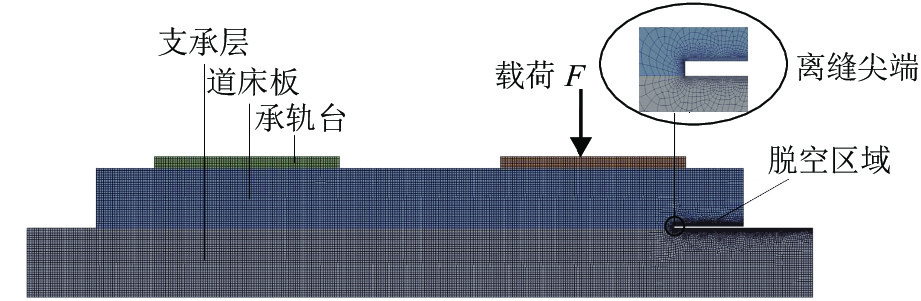
采用ANSYS Workbench建立无砟轨道层间离缝冒浆平面简化模型，网格划分如图1所示，对结构进行谐响应分析，荷载平均施加在承轨台上，荷载幅值取为钢轨支点力（扣件对轨道的作用力）的幅值[11]，荷载频率取25 Hz；在不考虑弹性基础的影响下，支承层底部采取全约束. 各部件计算参数如表1所示. 在此基础上，在脱空区域的最内侧（尖端）、中点、边缘处分别设置测点1、2、3，监测不同测点处的垂向相对位移（脱空区域道床板底面与支承层表面垂向位移差值），分析不同脱空深度和开口量对无砟轨道层间脱空区域变形特性的影响.
表 1 材料参数Table 1. Material parameters部件 强度 弹性模量/GPa 泊松比 密度/（kg·m−3） 轨枕 C60 35.5 0.20 2500 道床板 C40 32.5 0.20 2450 支承层 C15 22.0 0.18 2400 1.1 脱空深度的影响
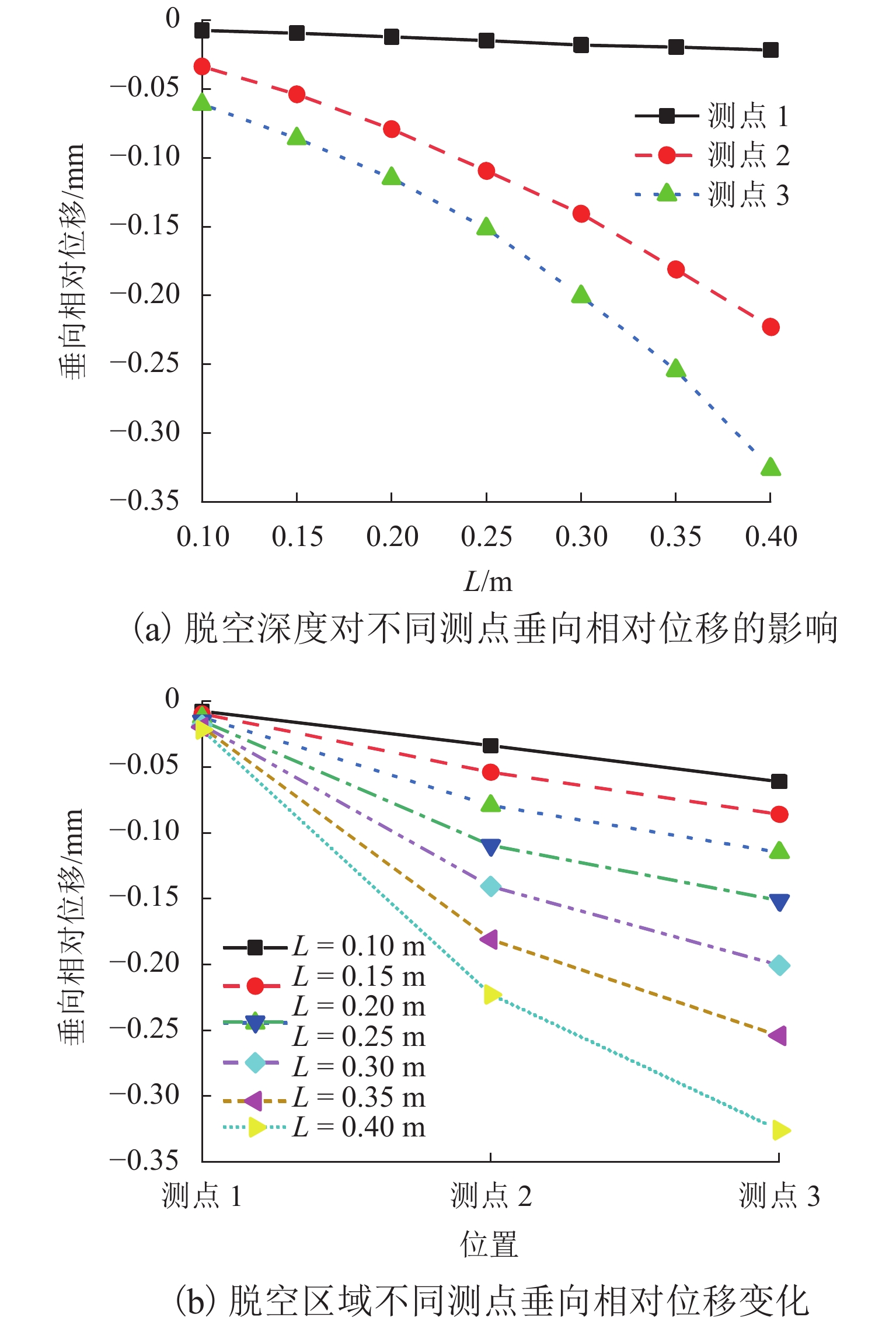
由于层间脱空区域扩展到承轨台下方时，会导致结构受力状态的改变，故首先分析不同脱空深度下脱空区域的变形行为. 当脱空深度L分别为0.10、0.15、0.20、0.25、0.30、0.35 m和0.40 m[10]，开口量h=8 mm时，提取不同监测点垂向相对位移，负值表示向下运动，如图2所示. 由图可知：随着脱空深度的增大，脱空区域不同位置垂向相对位移近似线性增大，在测点1处变化幅度很小，垂向相对位移接近于0；在测点3处变化幅度最大，脱空深度增大4倍，垂向相对位移增大4倍～5倍；在不同脱空深度下，脱空区域垂向相对位移在最内侧最小，在边缘处最大，脱空区域垂向相对位移沿脱空边缘方向近似线性增大.
图 2 不同脱空深度下脱空区域变形特性Figure 2. Deformation characteristic under different debonding depths1.2 开口量的影响
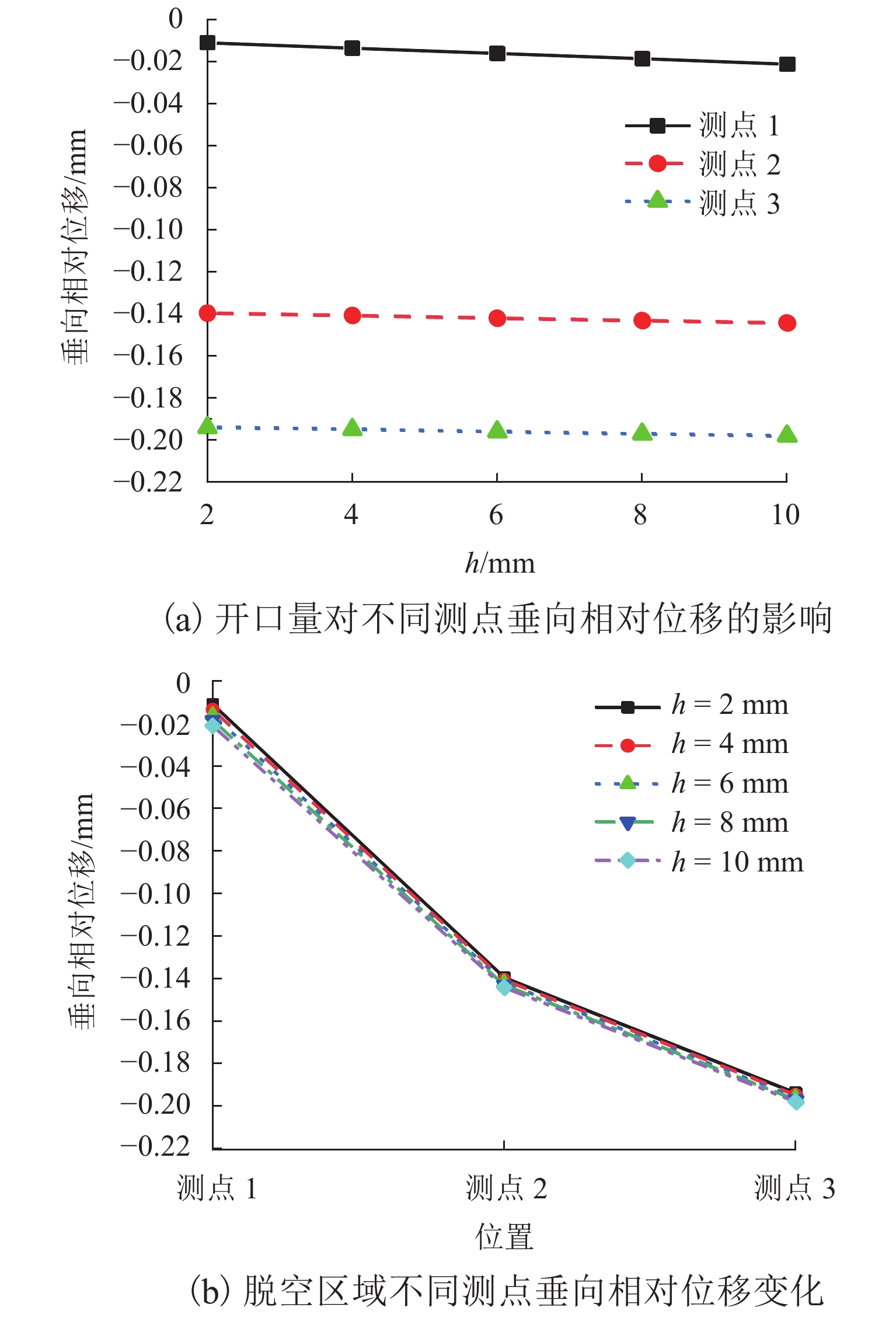
取L = 0.30 m，分析开口量对脱空区域变形行为的影响，h = 2，4，6，8，10 mm[12]，计算结果如图3所示. 由图3可知：随着开口量增大，脱空区域不同测点垂向相对位移近似相等，表明开口量的变化对脱空区域的变形行为影响很小；在不同开口量下，脱空区域垂向相对位移在最内侧最小，在边缘处最大，最大垂向相对位移均在0.220 mm内. 综合1.1、1.2节计算分析发现，当L = 0.30 m时，脱空区域最大垂向相对位移为0.201 mm，与文献[13]现场测试结果接近.
图 3 不同开口量下脱空区域变形特性Figure 3. Deformation characteristic under different crack openings2. 层间脱空模拟装置设计
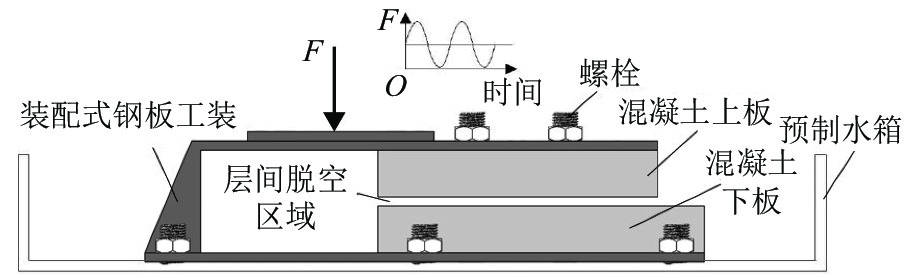
本文对轨道结构进行简化，将无砟轨道简化为道床板与支承层结构. 实际无砟轨道层间离缝形状是不规则的，假定离缝为规则的形状. 基于列车荷载作用下无砟轨道层间脱空区域的变形特性，结合课题组研制的高频加载装置，设计出如图4所示的模拟装置. 模拟装置由装配式钢板工装、预制水槽、高频加载装置、螺栓、混凝土板等构成，混凝土上、下板之间的空隙作为层间脱空区域. 模拟原理：装配式钢板工装的上板可视为悬臂结构，在高频荷载作用下，上板边缘垂向位移最大. 装配式钢板工装上下板通过螺栓与混凝土板联结，当钢板上板受力变形时，混凝土上板随之产生相同的位移，且保证混凝土边缘处的垂向位移最大，层间脱空区域垂向相对位移沿脱空边缘方向近似线性增大，符合列车荷载作用下脱空区域的变形特性.
根据第1节的计算分析，为模拟现场实际荷载作用下脱空区域的变形特征，设计层间脱空离缝深度为300 mm，宽度为200 mm，开口量在0～15 mm内调节. 混凝土上板尺寸为300 mm × 200 mm × 50 mm，混凝土下板尺寸为350 mm × 200 mm × 50 mm. 混凝土上、下板试件的强度等级为C40，试件内预埋螺栓与装配式钢板工装连接. 装配式钢板工装由10 mm的钢板焊接制作. 预制水槽尺寸为1600 mm × 800 mm × 150 mm. 水槽底面预埋6个螺栓，通过螺栓与装配式钢板工装连接，以固定结构，减少试验过程中结构振动对试验结果的影响. 高频加载装置放置到加载装置安置板上，荷载形式为正弦曲线，荷载频率可达50 Hz.
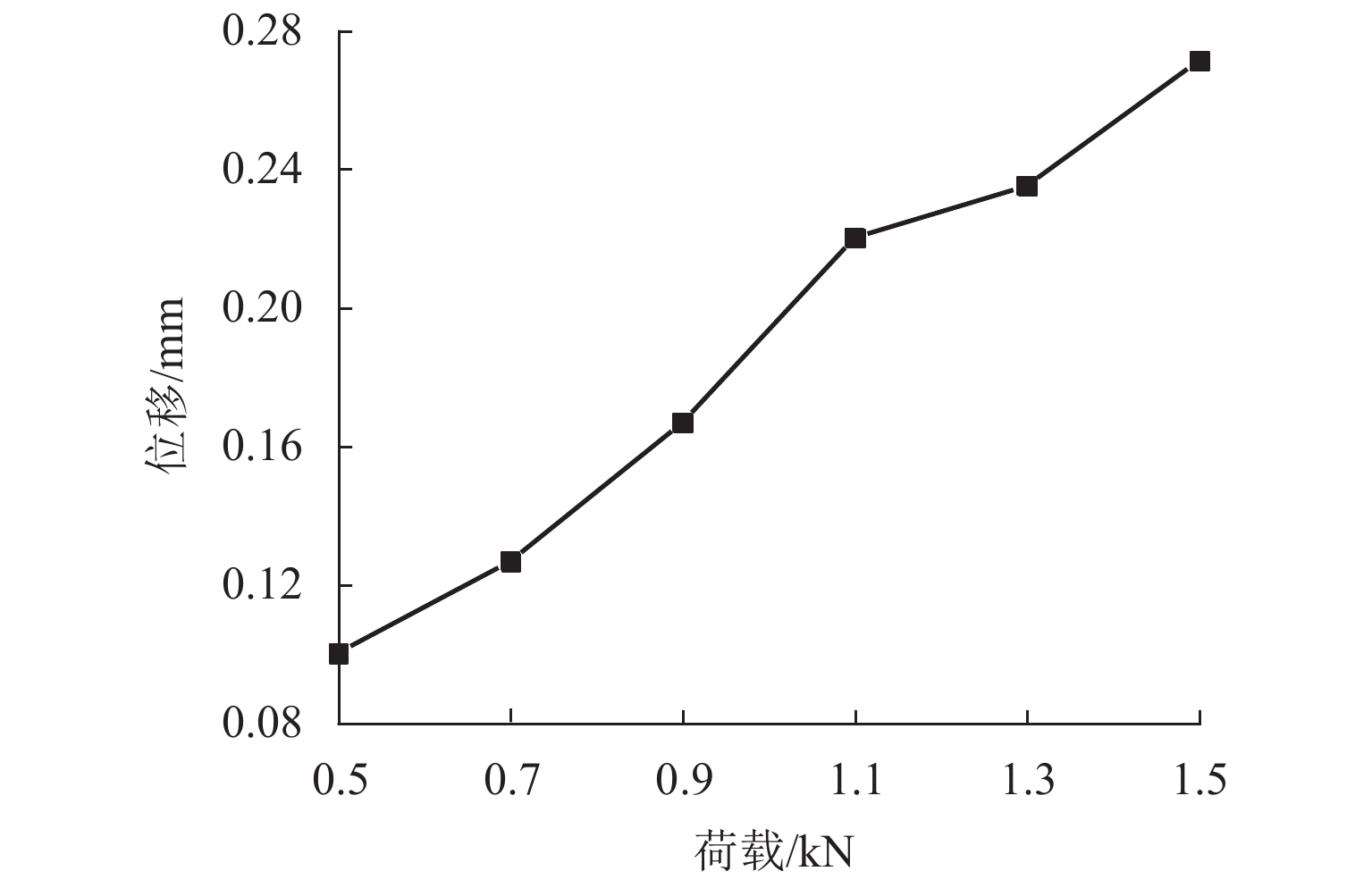
利用ANSYS Workbench对钢板工装进行谐响应分析，计算不同荷载作用下结构上板的垂向相对位移，与现场测试结果对比，确定试验实际加载值. 装置材料选用结构钢，弹性模量为 210.0 GPa，泊松比为 0.30. 装置底部设置为全约束，侧面约束纵向和横向位移. 当荷载频率为25 Hz，不同荷载幅值作用下结构上板边缘垂向相对位移如图5所示. 由图5可知，在荷载0.5～1.5 kN下，随着荷载增加，结构上板边缘位移近似线性增大，垂向相对位移值均在现场实测结果之内. 当荷载幅值为1.1 kN时，结构上板边缘垂向相对位移为0.220 mm，较为接近L = 0.30 m，h = 8 mm的工况下脱空区域边缘的垂向相对位移.
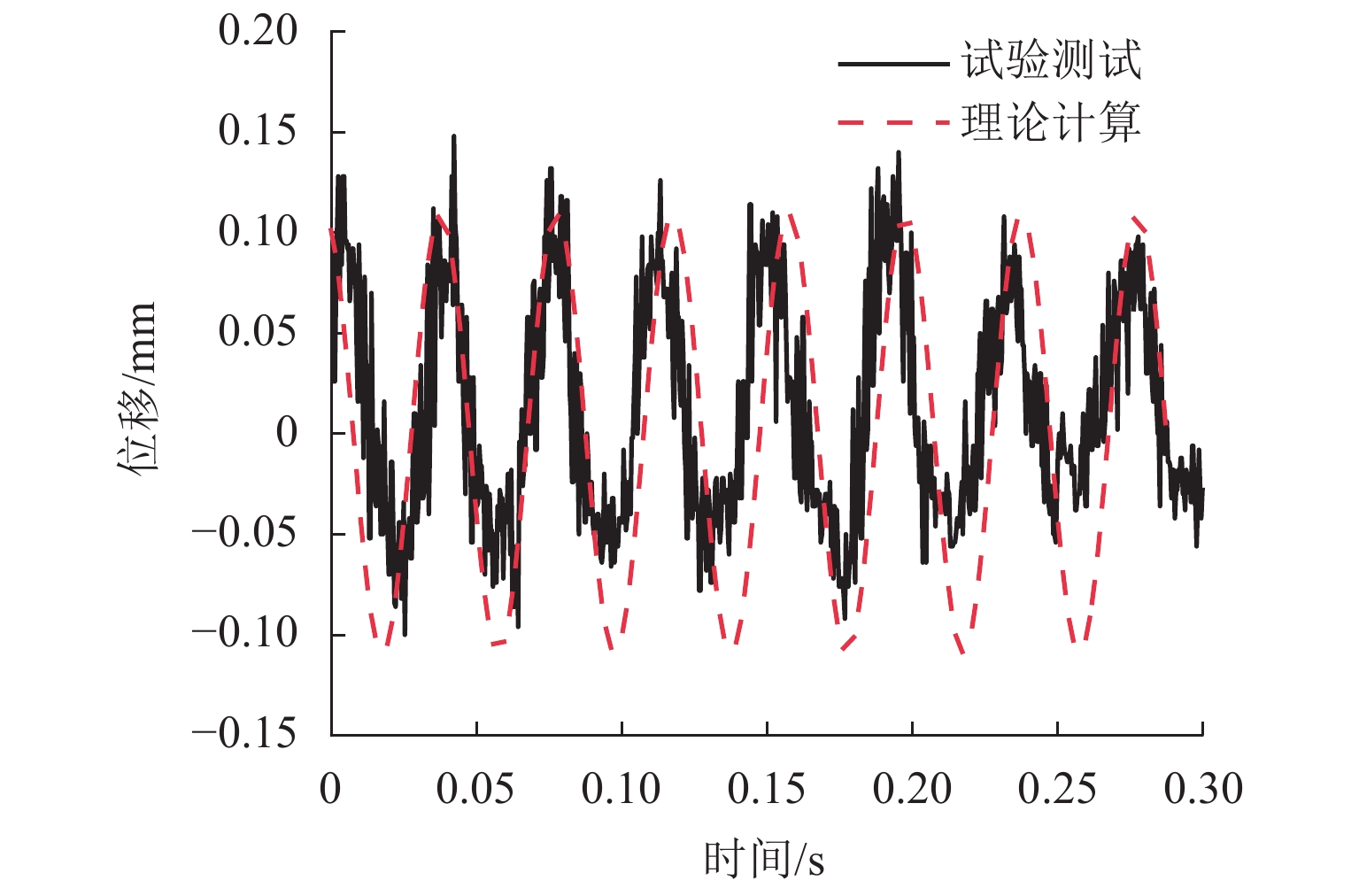
图 5 不同荷载作用下结构上板垂向相对位移Figure 5. Vertical relative displacements of the upper plate under different loads组装试验装置，在荷载幅值为1.1 kN、荷载频率为25 Hz的条件下，采用高精度接触式位移计测试板端位移，对比试验与理论计算结果，如图6所示. 在高频加载装置作用下，试验测试板端最大垂向相对位移约为0.20 mm，与理论计算结果0.22 mm接近，表明设计的层间脱空模拟装置可以用于模拟测试高频荷载作用下无砟轨道层间脱空区域的变形行为.
图 6 试验结果与理论计算结果对比Figure 6. Comparison of results between experimental and theoretical calculation3. 无砟轨道层间动水压力试验
3.1 试验概况
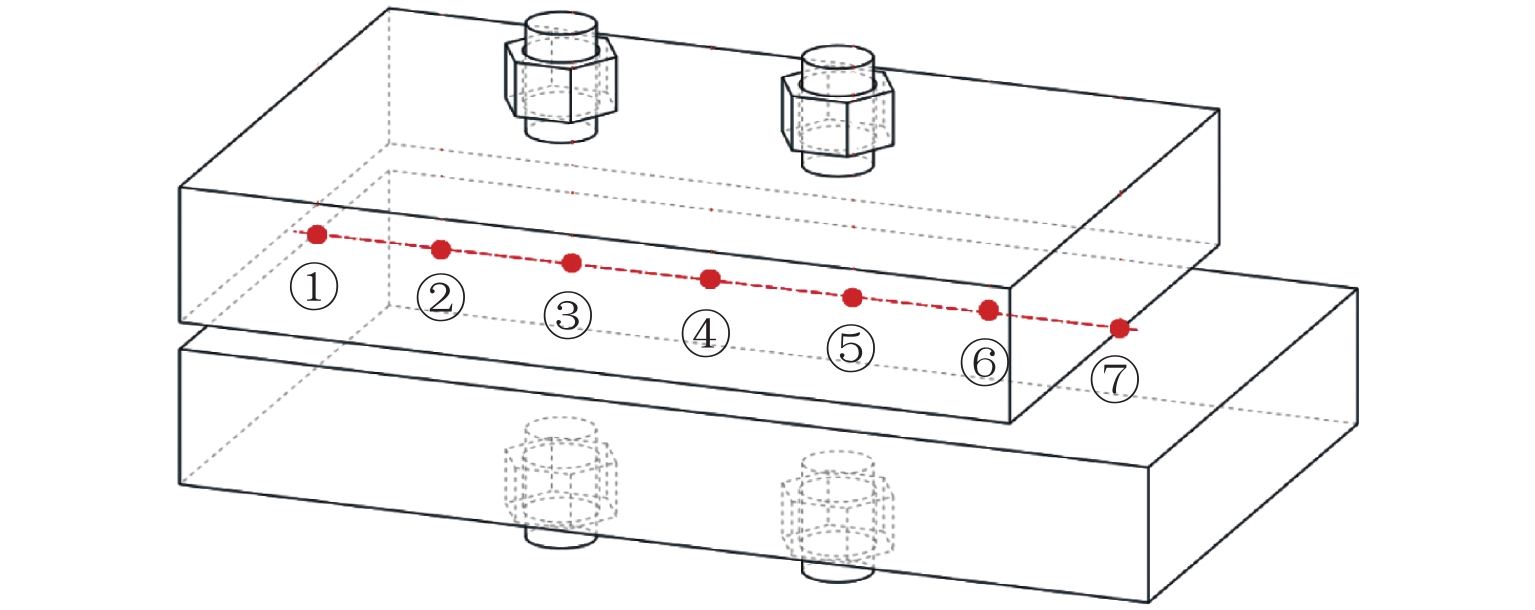
结合前文设计的层间脱空装置，开展无砟轨道层间离缝动水压力试验. 向预制水槽中注入20 ℃的自来水，保证水位高于离缝上表面. 层间脱空区域后部及两侧利用防水胶黏接，保证离缝内的水仅沿着出口方向流动. 压力传感器测试端通过软管连接皮托管深入离缝内部，另一端与采集软件Smart Sensor连接，实时监测测点水压力. 各个测点等间距分布，相邻测点间距为50 mm，测点 ① 位于层间离缝最内侧，测点 ⑦ 位于离缝开口处，具体测量位置如图7所示. 由于脱空区域变形特征与脱空深度有关，而且为了方便测试，适应压力传感器尺寸，试验时开口量取为8、14 mm. 按照表2的4种设计工况进行试验.
表 2 试验工况Table 2. Test working conditions试验工况 开口量/mm 加载频率/Hz 目的 1 8 15 加载频率对动水压力的影响 2 8 25 3 8 25 开口量对动水压力的影响 4 14 25 3.2 试验结果及分析
3.2.1 动水压力时间分布特性
在加载频率25 Hz、层间离缝开口量8 mm的工况，提取加载后的试验数据，分析不同监测点的动水压力时程分布规律，如图8所示. 在高频荷载作用下，不同测点动水压力变化趋势总体呈现正弦形式，其值正负交替变化，随着荷载达到最大值后达到峰值. 加载时，由于道床板混凝土底面靠近支承层混凝土表面，使得层间脱空区域内水被排出，此时脱空区域内产生正压力；卸载时，由于道床板混凝土底面远离支承层混凝土表面，使得层间脱空区域外水被吸回脱空区域内，此时脱空区域内产生负压力. 以测点 ①为例，最大正压为15.794 kPa，最大负压为−16.766 kPa，压力差为32.560 kPa.
图 8 不同测点动水压力时程曲线Figure 8. Time history curves of hydrodynamic pressure at different measuring points3.2.2 动水压力空间分布特性
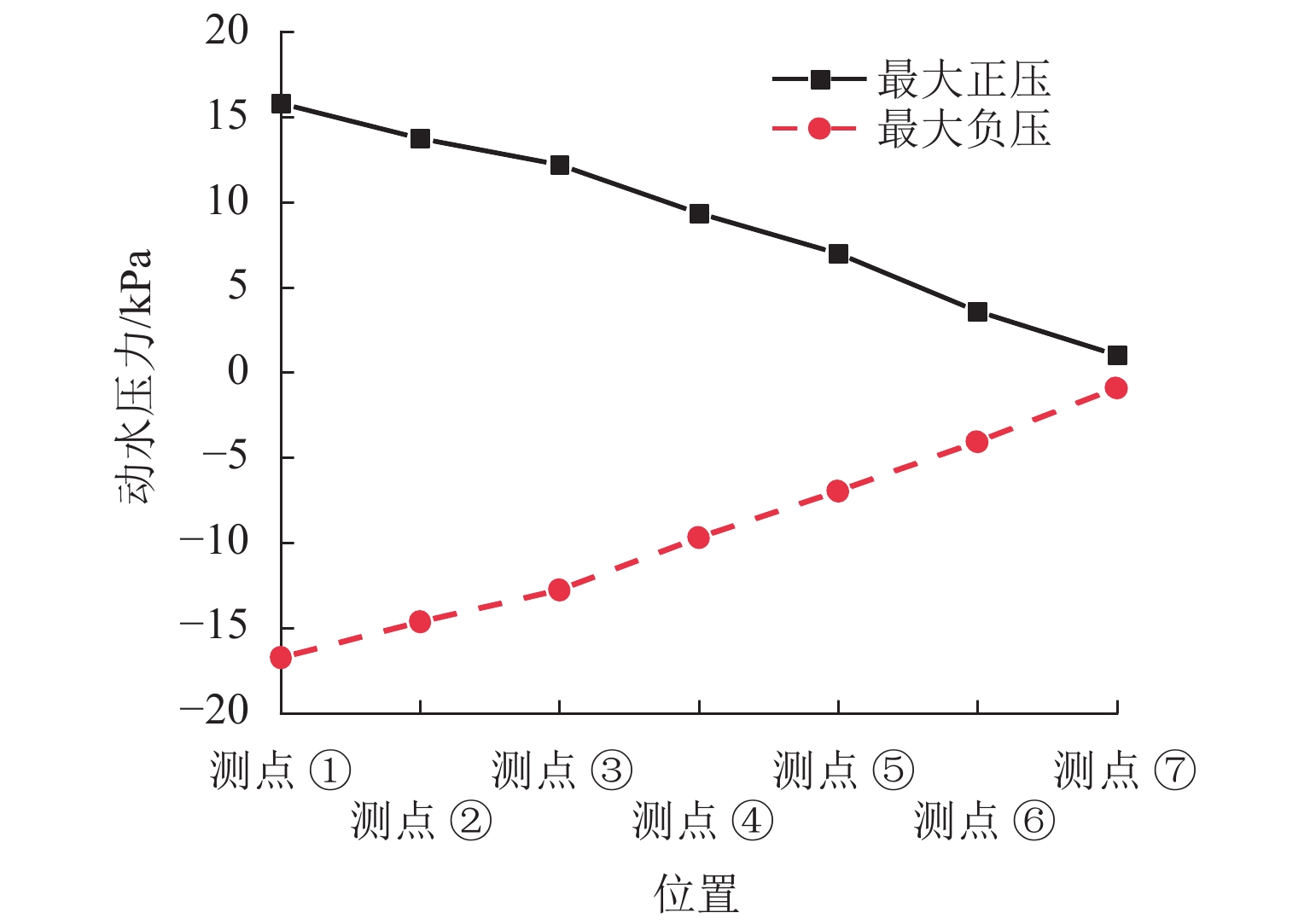
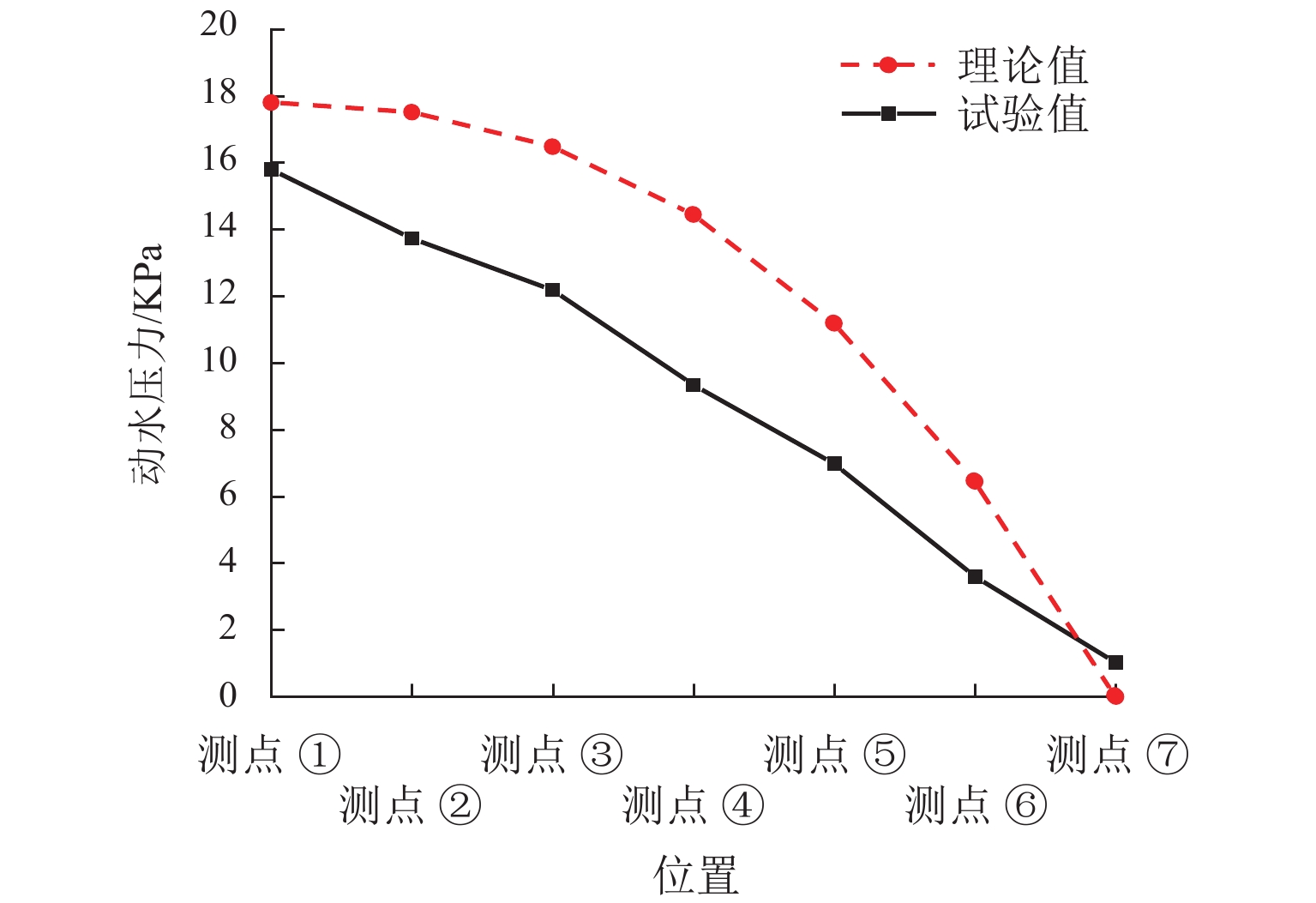
在加载频率25 Hz、层间离缝开口量8 mm的条件下，提取不同测点动水压力的最大正压和最大负压，如图9所示. 动水压力沿离缝长度方向近似线性分布，在测点 ① 处动水压力最大，监测点越靠近离缝出口，动水压力越小.
图 9 不同测点处动水压力最值Figure 9. Maximum values of hydrodynamic pressure at different measuring points3.2.3 加载频率对动水压力的影响
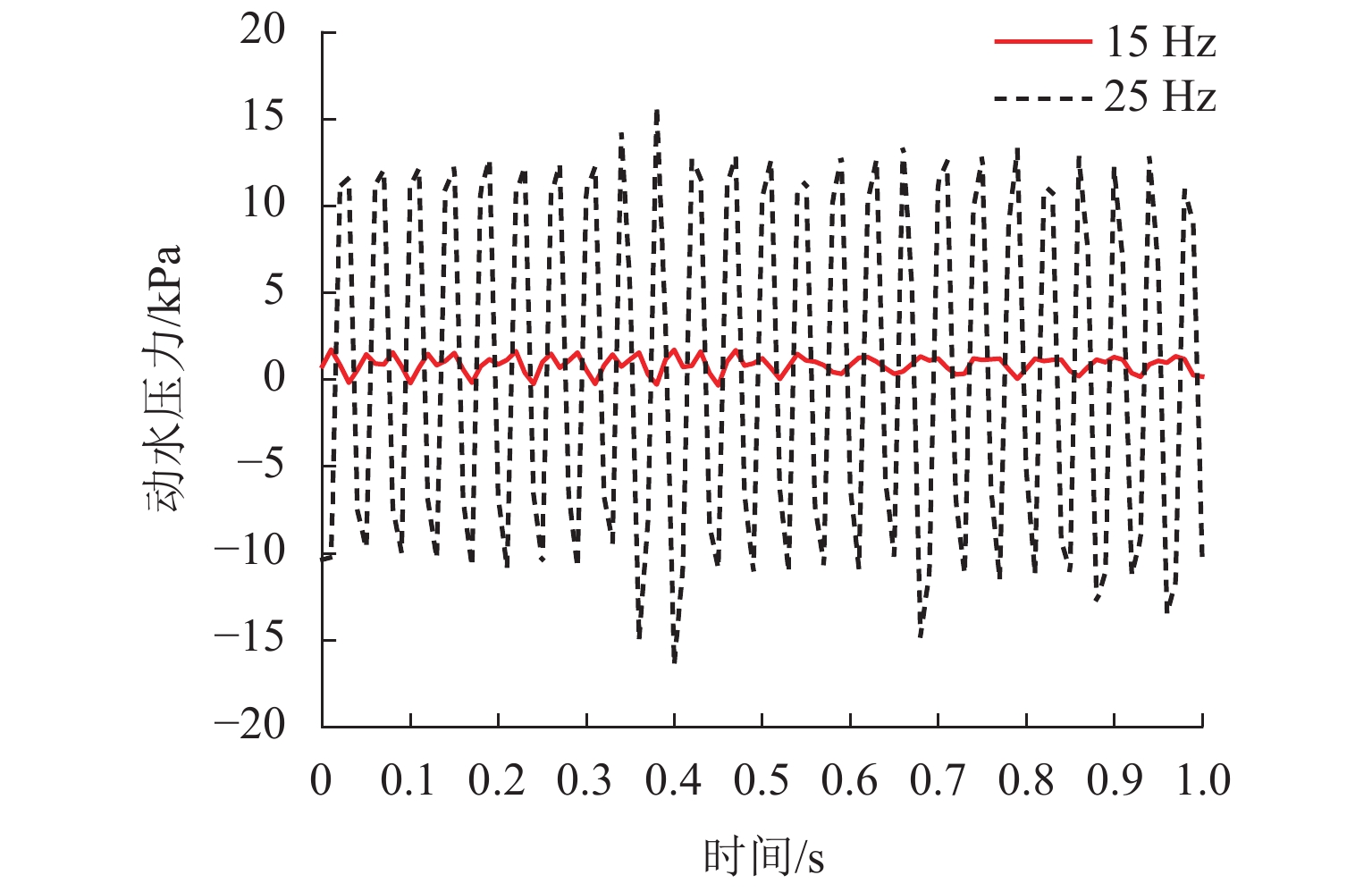
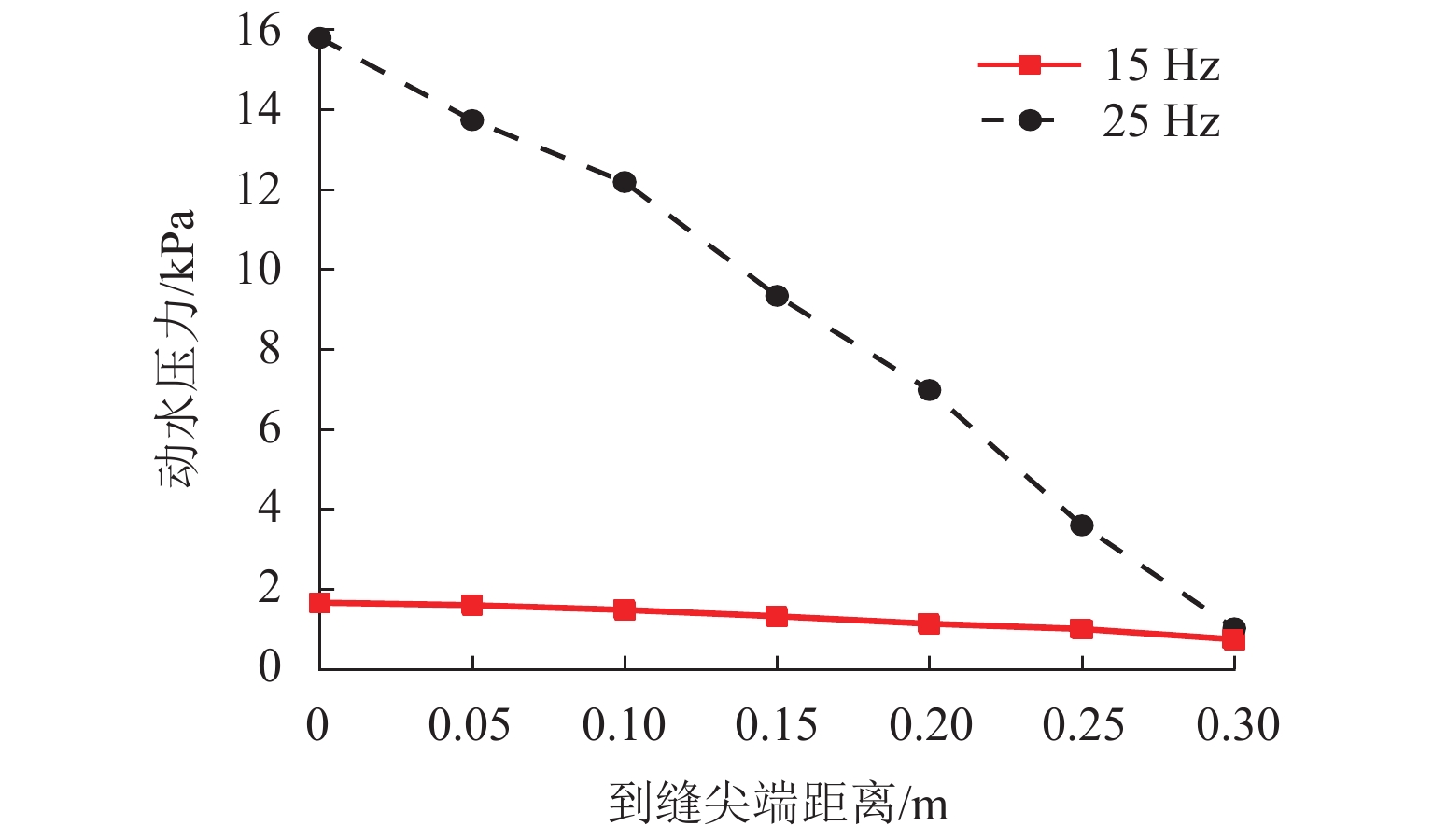
在离缝为8 mm的条件下改变加载频率大小，对比不同荷载频率下测点 ① 的动水压力时程曲线，如图10所示，当荷载频率为25 Hz时，该测点的最大动水压力约为15.794 kPa；当加载频率为15 Hz时，该处的最大动水压力约为1.646 kPa，频率增大10 Hz，最大动水压力增大接近10倍，说明荷载频率是无砟轨道层间离缝水压力的重要影响因素之一. 测得不同监测点动水压力沿离缝分布，如图11所示，随着加载频率增大，动水压力会大幅度增大. 在不同频率下，离缝内动水压力均在离缝深处最大，沿离缝出口方向减小.
图 10 不同加载频率下测点 ① 的动水压力时程曲线Figure 10. Time history curves of hydrodynamic pressure at measuring point ① with different loading frequencies3.2.4 离缝开口量对动水压力的影响
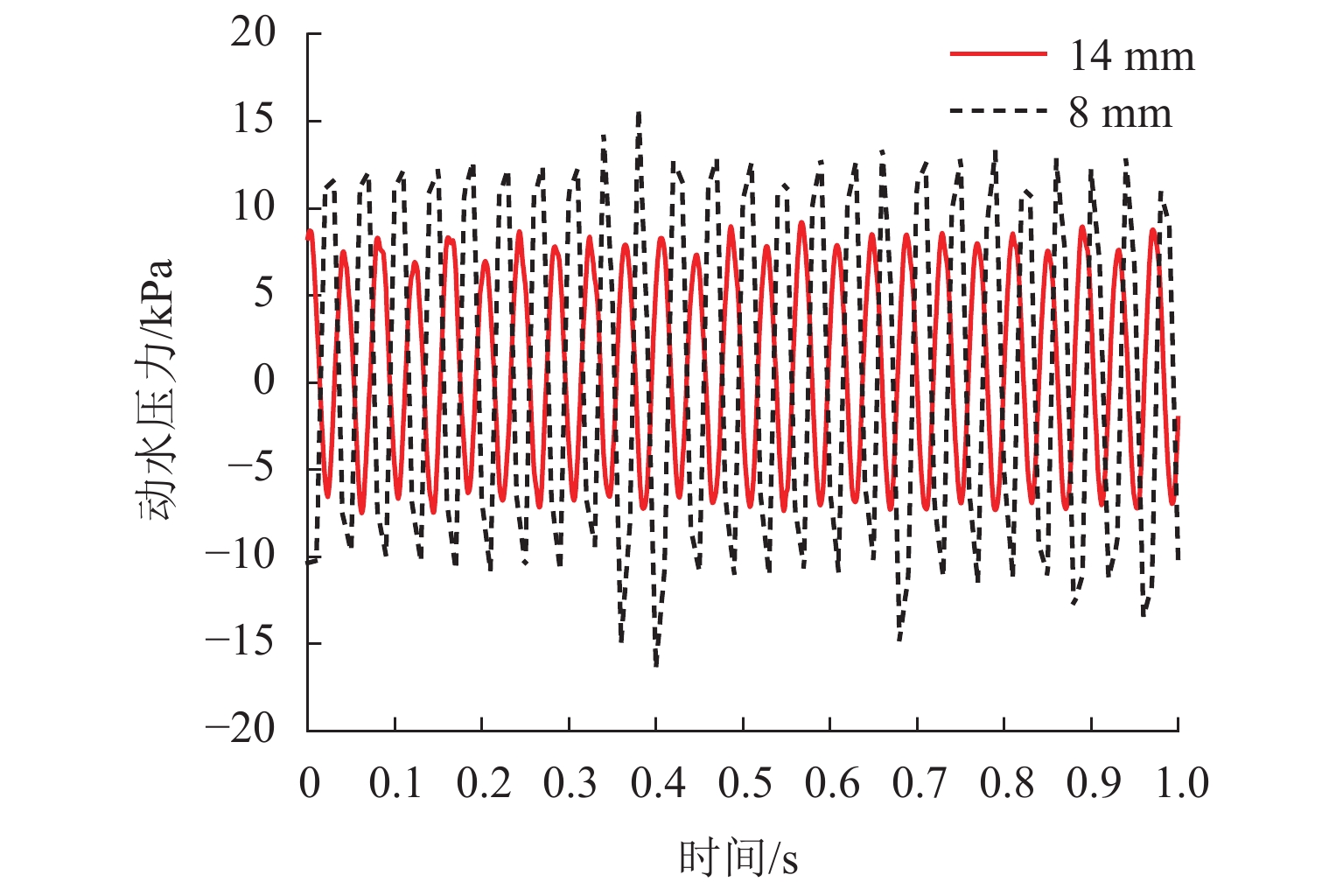
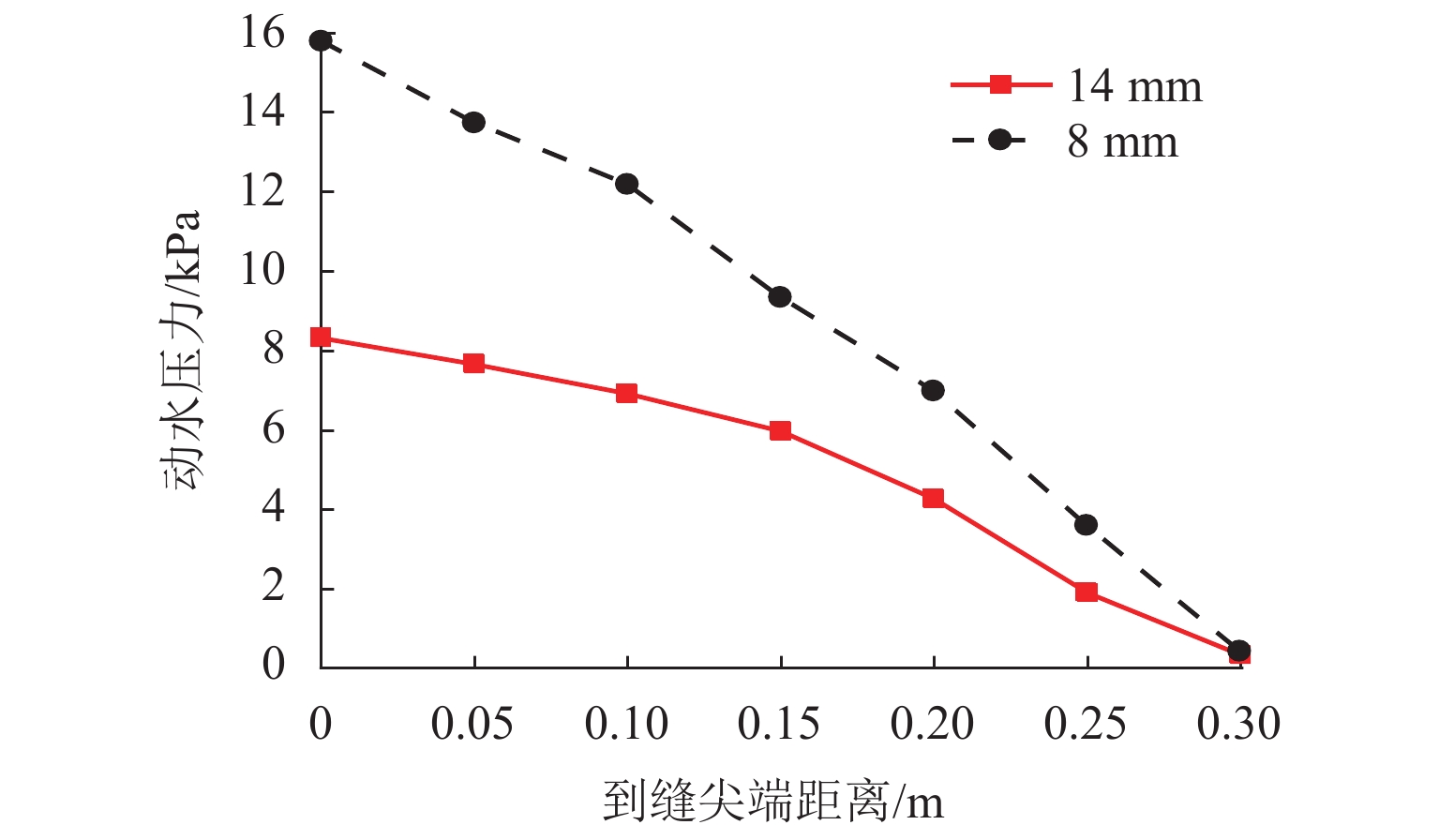
在加载频率为25 Hz时，对比不同离缝开口量下测点 ① 的动水压力时程曲线，如图12所示. 在开口量为8 mm时，测点 ① 的水压力为15.794 kPa；开口量为14 mm时，该处的水压力为8.320 kPa，说明离缝开口量是无砟轨道层间离缝水压力的重要影响因素之一. 测得不同监测点动水压力沿离缝分布如图13所示. 由图可知：随着离缝开口量的增大，动水压力会减小；在不同开口量下，离缝内动水压力均在离缝深处最大，沿离缝出口方向减小.
图 12 不同开口量下测点 ① 的动水压力时程曲线Figure 12. Time history curves of hydrodynamic pressure at measuring point ① with different crack openings3.3 试验模型验证
根据图4试验模型，利用ANSYS Workbench与CFX建立双向瞬态流固耦合模型，将层间脱空模拟装置和混凝土试件设置为固体域；混凝土层间脱空区域设为流体域，并假定水流状态为层流；将混凝土试件层间离缝上下表面设置为流固耦合面，便于固体域与流体域进行数据传递. 根据表1设置材料参数，计算相同荷载条件下（25 Hz）层间裂纹不同测点处的动水压力. 不同测点动水压力理论计算结果与试验结果对比如图14所示. 试验结果与理论计算结果存在一定误差，可能原因是试验过程存在局部水压力损失，但二者整体趋势接近，表明试验模型具有一定的有效性.
图 14 不同测点动水压力理论计算结果与试验结果对比Figure 14. Comparison of results between theoretical calculation and experiment of hydrodynamic pressure at different measuring points4. 结 论
1） 随着脱空深度L的增大，脱空区域垂向相对位移显著增大，但开口量h对脱空区域垂向相对位移影响很小. 当L = 0.3 m时，脱空区域最大垂向相对位移为0.201 mm与现场测试结果接近. 脱空深度L是影响脱空区域垂向相对位移的主要因素.
2） 当荷载频率为25 Hz，幅值为1.1 kN时，设计的层间脱空模拟装置板端最大垂向相对位移为0.220 mm，与现场测试结果接近，表明可以利用该装置模拟实际列车荷载作用下无砟轨道层间脱空区域水流特性.
3） 在高频荷载作用下，无砟轨道混凝土层间离缝内动水压力整体变化趋势呈现正弦形式，当荷载值达到最大时，离缝内部水压力达到峰值；相同条件下，离缝内动水压力在离缝深处最大，沿离缝出口方向减小. 当荷载频率为25、15 Hz时，层间离缝内最大动水压力分别为15.794、1.646 kPa，随着荷载频率增大，动水压力增大. 当离缝开口量为8、14 mm时，层间离缝内最大动水压力分别为15.794、8.320 kPa，随着开口量增大，动水压力减小.
致谢：西南交通大学原创性科研仪器项目（XJ2021KJZK035）的资助.
-
图 8 第i块加劲板动态配位系数示意
Figure 8. Illustration of dynamic coordination coefficient of i-th stiffened plate
图 12 加劲面积与临界荷载值的关系
Figure 12. Relationship between stiffening area and critical load value
图 13 加劲板数量与临界荷载的无量纲化关系
Figure 13. Dimensionless relationship between number of stiffened plates and critical load
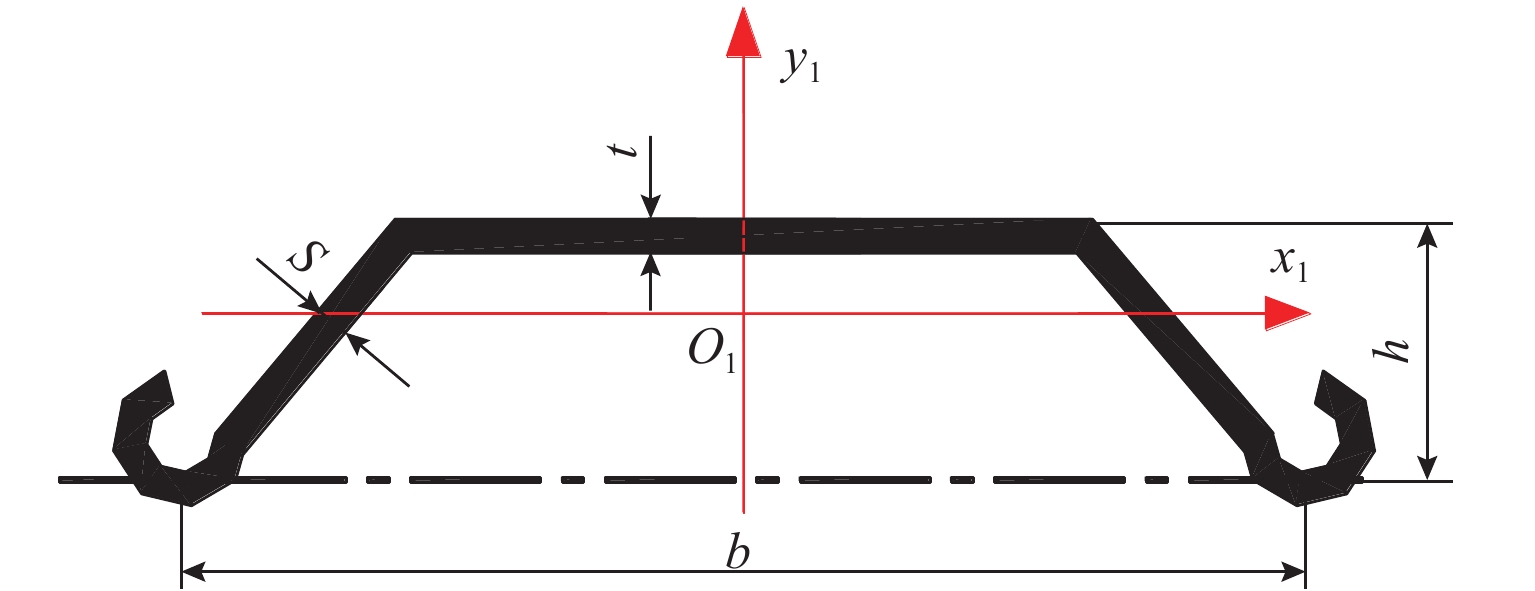
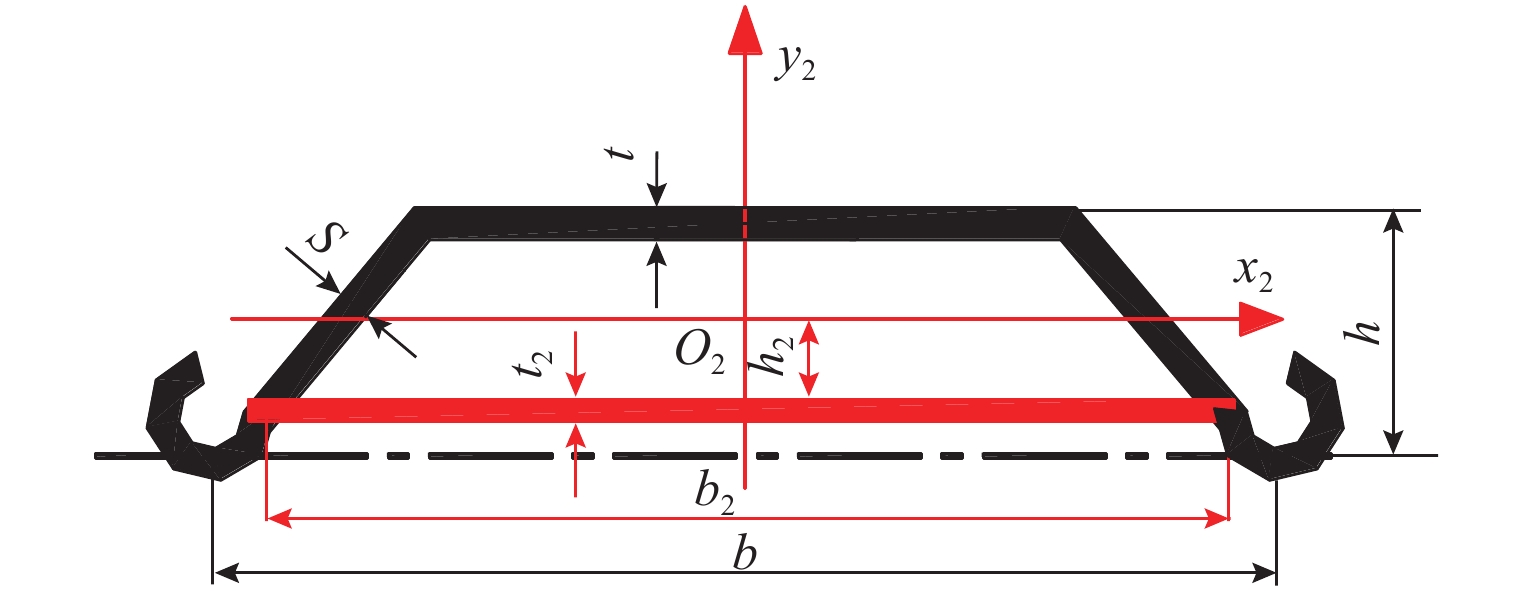
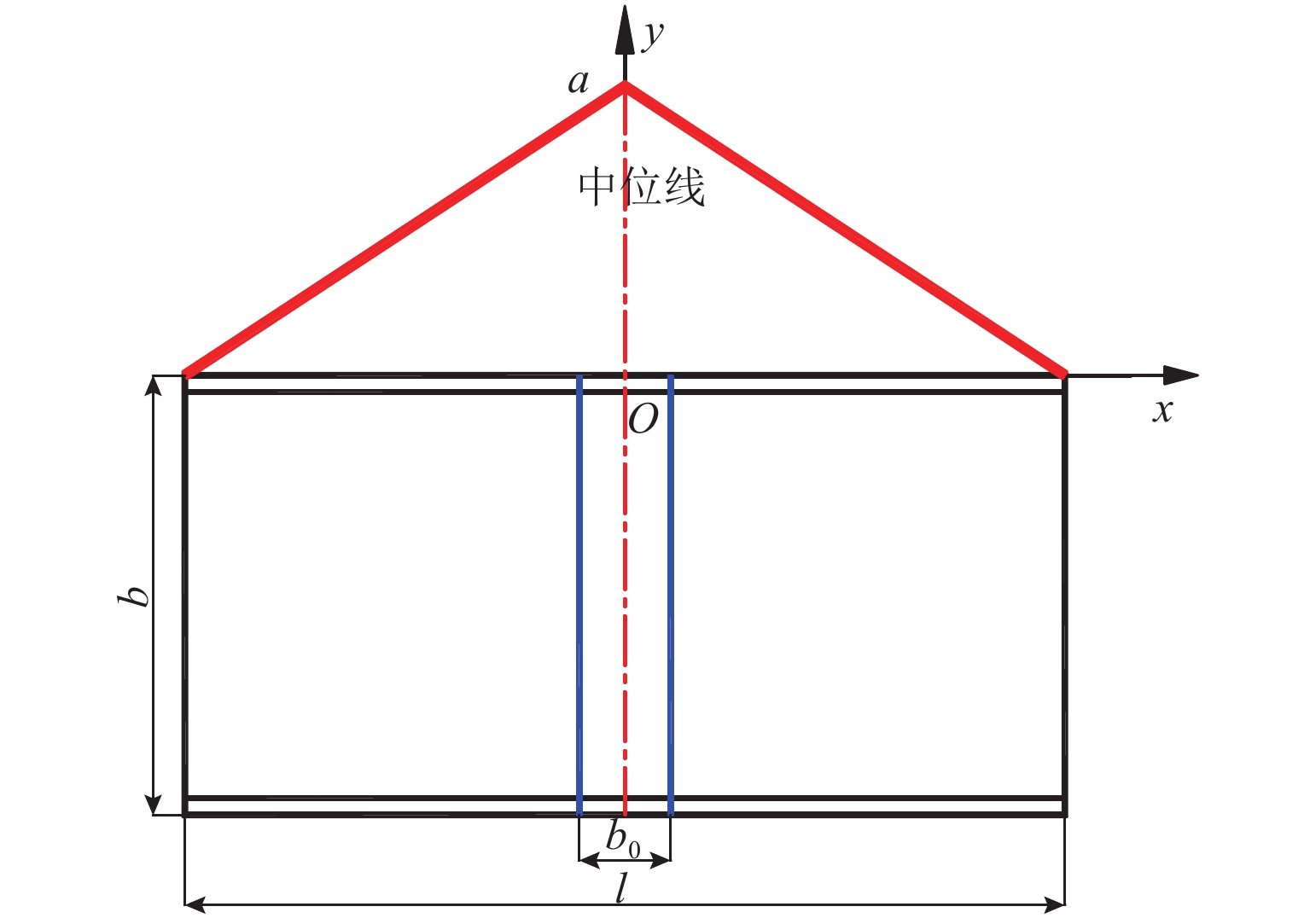
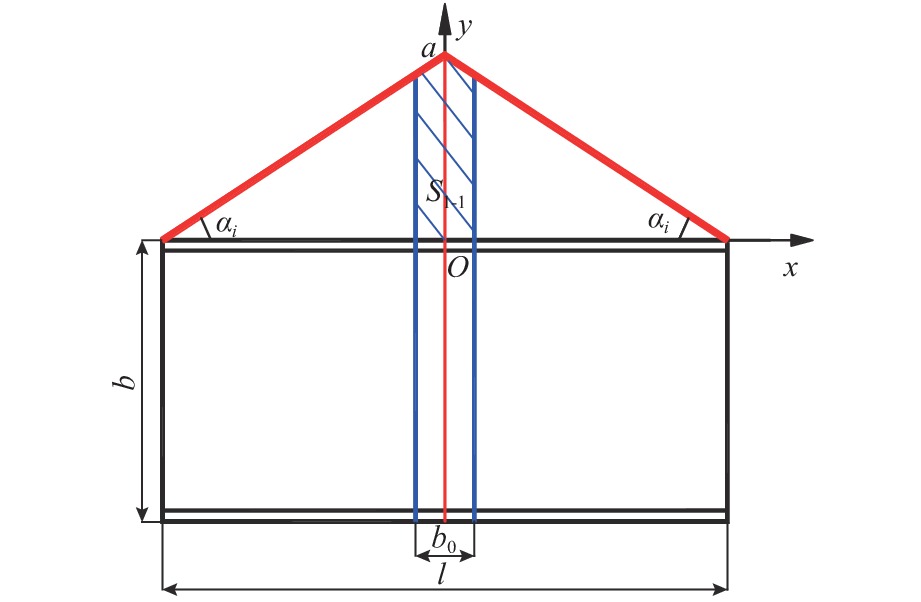
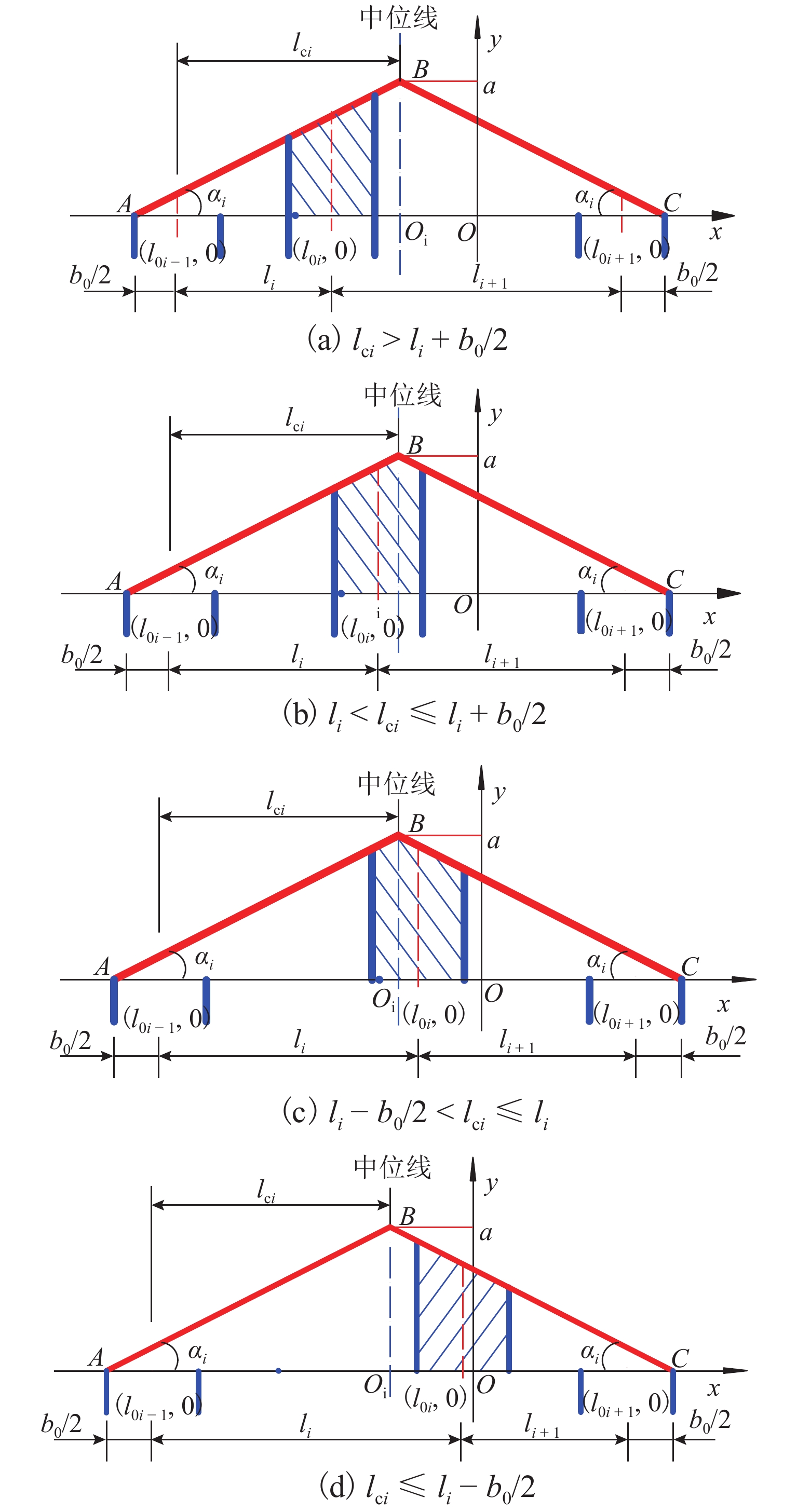
表 1 标准U型钢板桩及全长加劲下临界荷载
Table 1. Critical load of standard U-shaped steel sheet pile and U-shaped steel sheet pile under full-length stiffening
名称 l/m $\varphi $ Nmax/kN U 型钢板桩 10 0.502 1216.00 20 0.160 388.70 30 0.075 181.62 全长加劲 U 型钢板桩 10 0.480 1828.16 20 0.151 574.26 30 0.070 267.68 
下载: 导出CSV
表 2 20 m级构件临界荷载值最大化的加劲板布置优化方案
Table 2. Optimal arrangement of the number of stiffeners under different total stiffening areas for 20 m long members
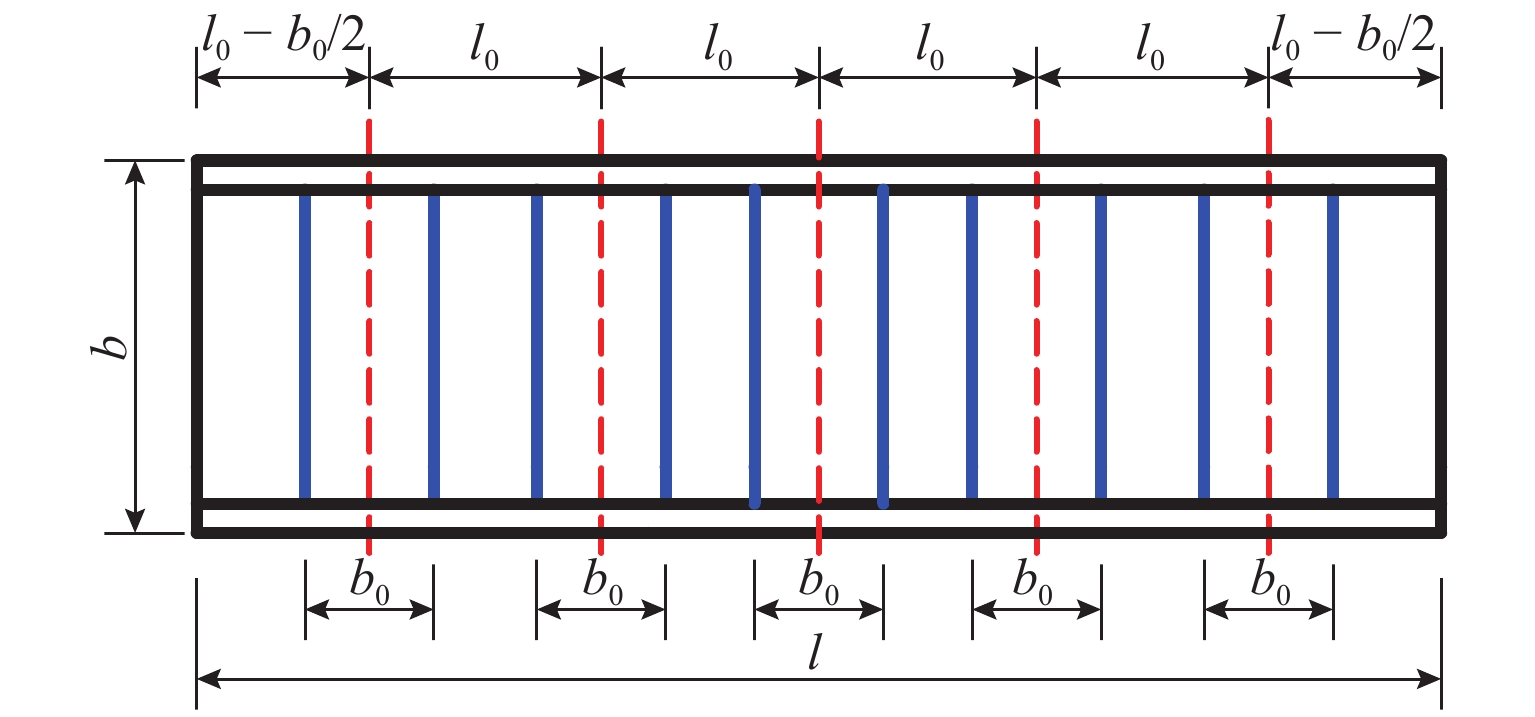
加劲总面积/m2 单根加劲板宽度/m 最优加劲方案 构件临界荷载值/kN [0,2.03）∪[14.18,15.00] [0,2.7）∪[18.9,20.00] 纵向对称轴处布置 1 根加劲板 [388.70,435.42）∪[573.70,574.26] [2.03,5.03）∪[13.35,14.18） [2.7,6.7）∪[17.8,18.9） 等间距布置 2 根加劲板 [435.42,494.29）∪[574.22,574.26] [5.03,6.15）∪[13.13,13.35） [6.7,8.2）∪[17.5,17.8） 等间距布置 3 根加劲板 [494.29,513.11）∪[574.25,574.26] [6.15,6.83）∪[13.05,13.13） [8.2,9.1）∪[17.4,17.5） 等间距布置 4 根加劲板 [513.11,523.51）∪574.26 [6.83,7.28）∪[12.90,13.05） [9.1,9.7）∪[17.2,17.4） 等间距布置 5 根加劲板 [523.51,530.05）∪574.26 [7.28,7.43） [9.7,9.9） 等间距布置 6 根加劲板 [530.05,532.15） [7.43,7.58） [9.9,10.1） 等间距布置 7 根加劲板 [532.15,534.23） [7.58,7.88） [10.1,10.5） 等间距布置 8 根加劲板 [534.23,538.26） [7.88,12.90） [10.5,17.2） 等间距布置 10 根加劲板 [538.26,574.26]
下载: 导出CSV
-
[1] SCHAFER B W. Local, distortional, and Euler buckling of thin-walled columns[J]. Journal of Structural Engineering, 2002, 128(3): 289-299. doi: 10.1061/(ASCE)0733-9445(2002)128:3(289) [2] LAU S C W, HANCOCK G J. Distortional buckling formulas for channel columns[J]. Journal of Structural Engineering, 1987, 113(5): 1063-1078. doi: 10.1061/(ASCE)0733-9445(1987)113:5(1063) [3] CAMOTIM D, DINIS P B. Coupled instabilities with distortional buckling in cold-formed steel lipped channel columns[J]. Thin-Walled Structures, 2011, 49(5): 562-575. doi: 10.1016/j.tws.2010.09.003 [4] MUTHURAMAN M, ANURADHA R, AWOYERA P O, et al. Numerical simulation and specification provisions for buckling characteristics of a built-up steel column section subjected to axial loading[J]. Engineering Structures, 2020, 207: 110256.1-110256.8. [5] ZHANG L L, TAN K H, ZHAO O. Local stability of press-braked stainless steel angle and channel sections: testing, numerical modelling and design analysis[J]. Engineering Structures, 2020, 203: 109869.1-109869.18. [6] 吕蕴龙,郭兵. 支吊架用卷边槽钢轴压构件的稳定性研究[J]. 建筑结构,2021,51(7): 85-90. doi: 10.19701/j.jzjg.2021.07.013LÜ Yunlong, GUO Bing. Study on stability of cold-formed lipped channel steel axial compression members for supports and hangers[J]. Building Structure, 2021, 51(7): 85-90. doi: 10.19701/j.jzjg.2021.07.013 [7] 王春刚,张壮南,张耀春. 冷弯薄壁斜卷边槽钢轴压构件承载力计算的直接强度法研究[J]. 工程力学,2012,29(3): 75-82.WANG Chungang, ZHANG Zhuangnan, ZHANG Yaochun. Direct strength method study on bearing capacity calculation of channel columns with inclined simple edge stiffeners under axial compression[J]. Engineering Mechanics, 2012, 29(3): 75-82. [8] 王子龙. 腹板V形加劲的冷弯卷边槽钢轴压下局部和畸变屈曲分析[D]. 哈尔滨: 哈尔滨工业大学, 2006. [9] 姚永红. 腹板V形加劲冷弯薄壁卷边槽钢轴压柱稳定性能研究[D]. 哈尔滨: 哈尔滨工业大学, 2012. [10] 胡启平,韩璐. 带缀板的开口薄壁钢构件整体稳定分析[J]. 江西建材,2015(9): 6-7. doi: 10.3969/j.issn.1006-2890.2015.09.005HU Qiping, HAN Lu. Overall stability analysis of open thin-walled steel members with battens[J]. Jiangxi Building Materials, 2015(9): 6-7. doi: 10.3969/j.issn.1006-2890.2015.09.005 [11] 王春刚,张壮南,张耀春. 中间加劲复杂卷边槽钢轴心受压构件承载力试验研究[J]. 工程力学,2013,30(1): 221-228,254. doi: 10.6052/j.issn.1000-4750.2011.05.0328WANG Chungang, ZHANG Zhuangnan, ZHANG Yaochun. Experimental investigation on channel columns with complex edge stiffeners and intermediate stiffeners under axial compression[J]. Engineering Mechanics, 2013, 30(1): 221-228,254. doi: 10.6052/j.issn.1000-4750.2011.05.0328 [12] 王春刚,张壮南,贾连光,等. 板件中间V形加劲复杂卷边槽钢轴压构件的稳定性能研究[J]. 工程力学,2012,29(增2): 113-116,121. doi: 10.6052/j.issn.1000-4750.2012.05.S022WANG Chungang, ZHANG Zhuangnan, JIA Lianguang, et al. Stability behavior research of channels with complex edge stiffeners and intermediate V type stiffeners under axial compression[J]. Engineering Mechanics, 2012, 29(S2): 113-116,121. doi: 10.6052/j.issn.1000-4750.2012.05.S022 [13] 王春刚, 徐子风, 刘泓, 等. 腹板加劲复杂卷边槽钢偏心受压构件稳定性分析[C]//第22届全国结构工程学术会议论文集第Ⅱ册. 乌鲁木齐: [出版者不详], 2013: 303-306. [14] 宋波,王连广,王春刚. 帽形加劲复杂卷边槽钢柱的稳定性能[J]. 东北大学学报(自然科学版),2018,39(1): 143-147. doi: 10.12068/j.issn.1005-3026.2018.01.029SONG Bo, WANG Lianguang, WANG Chungang. Stability behavior of channel columns with complex edge stiffeners and cap shaped stiffeners[J]. Journal of Northeastern University (Natural Science), 2018, 39(1): 143-147. doi: 10.12068/j.issn.1005-3026.2018.01.029 [15] 姜亦鑫. 支吊架用卷边槽钢压弯构件的整体稳定性计算[J]. 计算机辅助工程,2021,30(2): 50-55. doi: 10.13340/j.cae.2021.02.009JIANG Yixin. Global stability calculation on lipped channel compression flexural member for support and hanger[J]. Computer Aided Engineering, 2021, 30(2): 50-55. doi: 10.13340/j.cae.2021.02.009 [16] 陈明,周绪红,刘占科,等. 腹板加强型冷弯薄壁卷边槽钢柱受压性能试验研究[J]. 建筑结构学报,2017,38(2): 37-47. doi: 10.14006/j.jzjgxb.2017.02.005CHEN Ming, ZHOU Xuhong, LIU Zhanke, et al. Experimental investigation of web-stiffened cold-formed lipped channel columns[J]. Journal of Building Structures, 2017, 38(2): 37-47. doi: 10.14006/j.jzjgxb.2017.02.005 [17] 孙觅. 加隔板的冷弯薄壁卷边槽钢偏心受压性能试验研究与有限元分析[D]. 杭州: 浙江大学, 2008. [18] 杨文斌. 高强冷弯薄壁卷边槽钢轴心受压稳定性能研究[D]. 大连: 大连理工大学, 2020. [19] 顾建飞,姚谏,钱国桢. 冷弯薄壁槽钢柱畸变屈曲的有限元分析及一种控制措施[J]. 科技通报,2007,23(1): 111-115. doi: 10.3969/j.issn.1001-7119.2007.01.022GU Jianfei, YAO Jian, QIAN Guozhen. Finite element analysis and an effective measure to distortional buckling of cold-formed channel columns[J]. Bulletin of Science and Technology, 2007, 23(1): 111-115. doi: 10.3969/j.issn.1001-7119.2007.01.022 [20] 李元齐,刘翔,沈祖炎,等. 高强冷弯薄壁型钢卷边槽形截面轴压构件畸变屈曲控制试验研究[J]. 建筑结构学报,2010,31(11): 10-16. doi: 10.14006/j.jzjgxb.2010.11.002LI Yuanqi, LIU Xiang, SHEN Zuyan, et al. Experimental study on distortional buckling control for high-strength cold-formed thin-walled steel lipped channel columns subject to axial compression[J]. Journal of Building Structures, 2010, 31(11): 10-16. doi: 10.14006/j.jzjgxb.2010.11.002 [21] 中华人民共和国建设部. 钢结构设计规范: GB 50017—2003[S]. 北京: 中国计划出版社, 2003. [22] 欧领特. 钢板桩工程手册[M]. 北京: 人民交通出版社, 2011. 期刊类型引用(2)
1. 元强，向功坤，郑新国，张旭，王勇. CRTS Ⅱ型板式轨道砂浆充填层服役现状分析. 铁道学报. 2025(02): 152-159 . 
百度学术2. 杨荣山，林航，刘珀全，牛鑫宇，陈健，谭斌. 无砟轨道层间颗粒冲刷试验研究. 中国铁道科学. 2024(06): 81-90 . 百度学术其他类型引用(1)
-
下载:
下载:
百度学术
点击查看大图
计量
- 文章访问数: 309
- HTML全文浏览量: 192
- PDF下载量: 21
- 被引次数: 3