连续排水边界下抽水引发的弱透水层固结解

江留慧; 李传勋; 吴文兵; 梅国雄

doi:10.3969/j.issn.0258-2724.20220473

连续排水边界下抽水引发的弱透水层固结解

doi: 10.3969/j.issn.0258-2724.20220473

1.
江苏大学土木工程与力学学院，江苏镇江 212013
2.
中国地质大学工程学院，湖北武汉 430074
3.
浙江大学海洋学院，浙江舟山 316021

基金项目: 国家自然科学基金项目（51878320）

详细信息

作者简介:
江留慧（1994—），女，实验师，硕士，研究方向为岩土工程，E-mail：jlh134606@163.com

通讯作者:
梅国雄（1975—），男，教授，博士，研究方向为岩土工程，E-mail：meiguox@163.com

中图分类号: TU46
计量
- 文章访问数: 266
- HTML全文浏览量: 111
- PDF下载量: 43
- 被引次数: 0
出版历程
- 收稿日期: 2022-07-05
- 修回日期: 2022-11-20
- 网络出版日期: 2023-12-04
- 刊出日期: 2022-11-25

Consolidation Solution of Aquitard Induced by Dropping of Groundwater Table with Continuous Drainage Boundary

1.
Faculty of Civil Engineering and Mechanics, Jiangsu University, Zhenjiang 212013, China
2.
Faculty of Engineering, China University of Geosciences, Wuhan 430074, China
3.
Ocean College, Zhejiang University, Zhoushan 316021, China

摘要

摘要:
为探究更能反映实际透水能力的排水边界对超采地下水导致的地面沉降问题的影响，首先，通过引入孔压随时间指数衰减的连续排水边界，建立连续排水边界下潜水层水位变化引发的下卧弱透水层一维固结模型；其次，采用分离变量法获得其模型普遍解析解答以及水位瞬时下降和水位单极等速下降2种特殊降水模式的解析解；然后，通过特定条件下解析解的退化初步验证本文解析理论的正确性；最后，以水位单级等速下降为例，利用本文解计算不同界面参数下弱透水层的固结曲线，着重分析界面参数对固结性状的影响. 结果表明：理论计算的沉降曲线与室内试验沉降曲线对比，最大误差为13%，进一步说明连续排水边界更贴合实际透水边界；界面参数越大（排水边界透水性越好），孔压消散速率越快，固结速率越快，越早完成固结，但其并不影响最终固结状态.
- 水位下降 /
- 土力学 /
- 连续排水边界 /
- 界面参数 /
- 解析解
Abstract:
In order to investigate the influence of the drainage boundary, which can better reflect the actual permeability, on the land subsidence caused by over-exploitation of groundwater, firstly, a one-dimensional consolidation model of aquitard caused by the dropping of groundwater table in phreatic aquifer with continuous drainage boundary was established by introducing the continuous drainage boundary, where the pore pressure decayed exponentially with time. Secondly, the general analytical solutions of the model and the analytical solutions of two special dewatering models for the instantaneous dropping of the groundwater table and the single-stage and constant-rate dropping of the groundwater table were obtained by using the method of separate variables. Then, the correctness of the analytical theory in this paper was preliminarily verified by the degeneracy of the analytical solutions under certain conditions. Finally, the single-stage and constant-rate dropping of the groundwater table was taken as an example, and the consolidation curves of the aquitard under different interface parameters B were calculated based on the analytical solutions. In addition, the influence of interface parameter B on the consolidation behaviors was analyzed emphatically. The results show that the maximum error rate is 13% by comparing the theoretically calculated settlement curve with the experimental settlement curve, which further indicates that the continuous drainage boundary is closer to the actual permeable boundary. Furthermore, larger interface parameter B indicates (better water permeability of the drainage boundary) a faster pore pressure dissipation rate, faster consolidation rate, and shorter consolidation time, but the final consolidation state is not affected.
- dropping of groundwater table /
- soil mechanics /
- continuous drainage boundary /
- interface parameter /
- analytical solution

HTML全文

过度开采地下水引起的地面沉降作为一种多发且持续时间长的环境地质灾害，严重阻碍着社会与经济的可持续发展^[1-3]. 如何正确预测地面沉降发展和科学防治地下水位变化引发的地面沉降灾害已成为学术界重要的课题之一. 能便捷预测地面沉降发展规律的解析解具备较强的实际工程应用潜力，因此，学者们相继对抽降水引发的地面沉降问题展开了固结解析解研究.

自太沙基建立一维固结理论以来，荷载作用下的土体固结问题已得到较为深入的研究. 与荷载作用引起固结沉降机理不同，在含水层抽降水引起水位下降，抽水层与相邻弱透水层之间的水压梯度差会诱发弱透水层释水，在此过程中有效应力的改变引起土体发生固结变形，最终表现为地面发生沉降^[4]. 基于太沙基一维固结理论，骆冠勇等^[5]获得了承压层瞬时减压引发的上覆弱透水层一维固结解析解. 谢康和等^[6]给出了承压层水位瞬时下降诱发的上覆弱透水层一维固结解析解. 黄大中^[7]分别推导了潜水层、承压层水位单级等速下降模式下弱透水层一维线性、非线性C_c/C_k=1 （C_c为压缩指数，C_k为渗透指数）固结解析解. 谢海澜等^[8]以孔隙渗流为非达西流为基础，获得了含水层降水引发的考虑土体的非达西渗流特性的弱透水层固结半解析解. 张玮鹏等^[9]考虑起始比降，获得了承压层大面积瞬时抽水引发的有起始比降的弱透水层一维固结解析解. 晏凌晗^[10]基于四元件线性流变模型，分别给出了潜水层水位瞬时下降和逐渐下降下弱透水层一维流变固结解析解. 吴浩等^[11]考虑结构性，推导了承压层水位单级等速下降引发的结构性弱透水层一维固结解析解. 夏长青^[12]考虑成层性，分别给出了潜水层和承压层水位单级等速下降引发的成层弱透水层一维固结解析解. 李传勋等^[13]考虑非线性压缩、渗透特性，推导了潜水层水位下降引发的弱透水层一维非线性（C_c/C_k≠1）固结解析解. 上述解析解研究分别考虑了弱透水层软黏土的成层性、流变性、非达西渗流、结构性、非线性等特性，但均将其排水边界视为完全透水边界或者完全不透水边界2种极端边界. 事实上，对于弱透水层这类透水性不良的土体，其透水边界排水能力介于完全透水和完全不透水之间.

梅国雄等^[14]提出了一种介于完全透水和完全不透水之间的连续排水边界，并给出了瞬时加载下考虑连续排水边界的土体一维固结解析解. 该排水边界将边界孔压考虑为随时间指数衰减，既能严格满足固结模型的初始条件，又可在一定条件下退化为完全透水边界. 基于此，冯健雪等^[15]推导了单级加载下考虑连续排水边界的土层一维固结解析解. 宗梦繁等^[16-17]先后分别考虑土体的非线性和流变性，推导了在连续排水边界下土体一维非线性固结解析解和一维流变固结解析解. 陈余等^[18]考虑起始水力坡降，推导了连续排水边界下考虑起始坡降的软黏土一维固结解析解. 张云鹏等^[19]综合考虑吹填土自重和吹填地基成层特性，推导了连续排水边界下成层陆域吹填地基一维固结解析解. 苗青等^[20]综合考虑土体非线性和土体的温度效应，获得了连续排水边界下考虑土体非线性的一维热固结问题的解析解. 但上述关于连续排水边界的固结理论研究均是基于外荷载作用展开的，目前对连续排水边界下抽降水引发的弱透水层固结理论研究的相关报道仍较少.

目前，对于越流系统中弱透水层固结理论的研究普遍是将土体边界视为完全透水或完全不透水，这样的理想情况与实际工况并不相符. 相对而言，边界排水能力与超静孔压相关，且随时间指数衰减的连续排水边界更为符合实际. 故十分有必要将弱透水层排水边界考虑为连续排水边界，对含水层水位下降诱发的弱透水层一维固结理论展开深入研究. 本文建立连续排水边界条件下潜水层大面积抽降水引发的下卧弱透水层一维固结模型，并获得其解析解答，着重分析界面排水系数b （无量纲界面参数B）对固结性状的影响. 本文提供的固结模型解答不仅完善了弱透水层固结理论体系，而且对弱透水层的沉降计算具有更广泛的适用性.

1. 固结模型的建立

1.1 问题描述

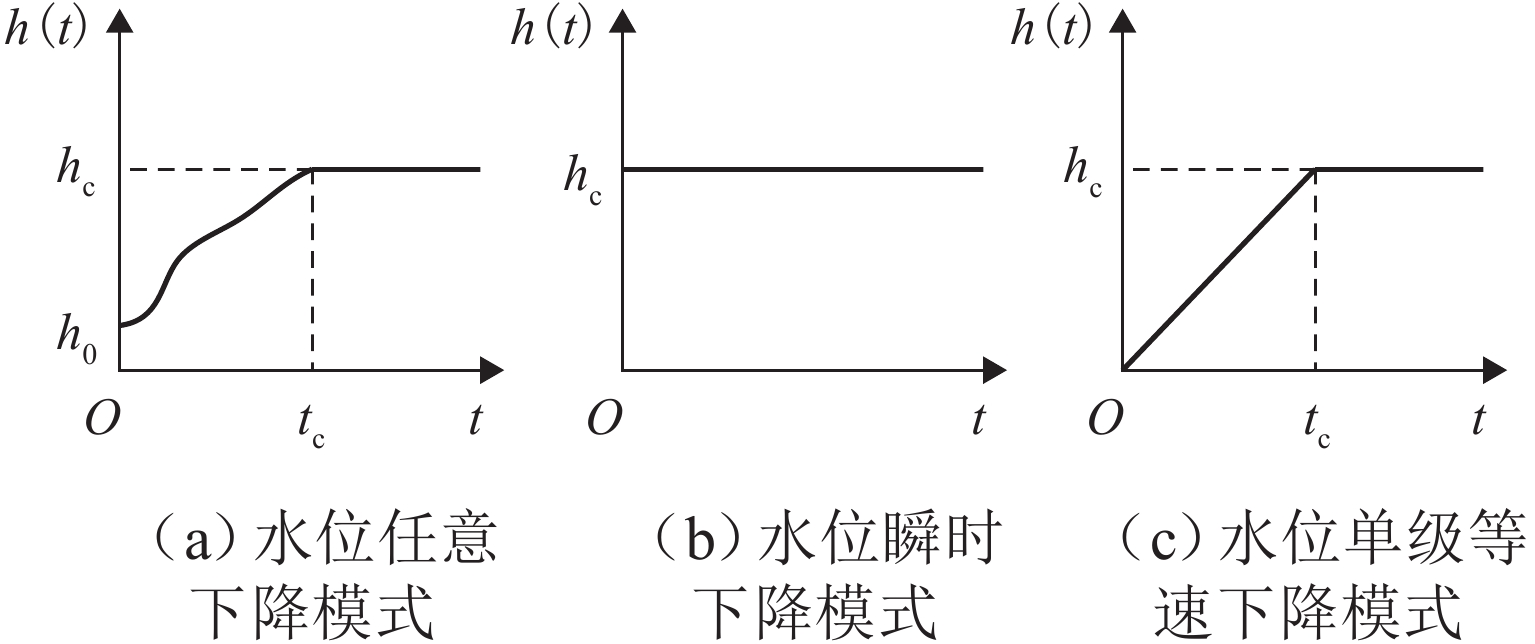
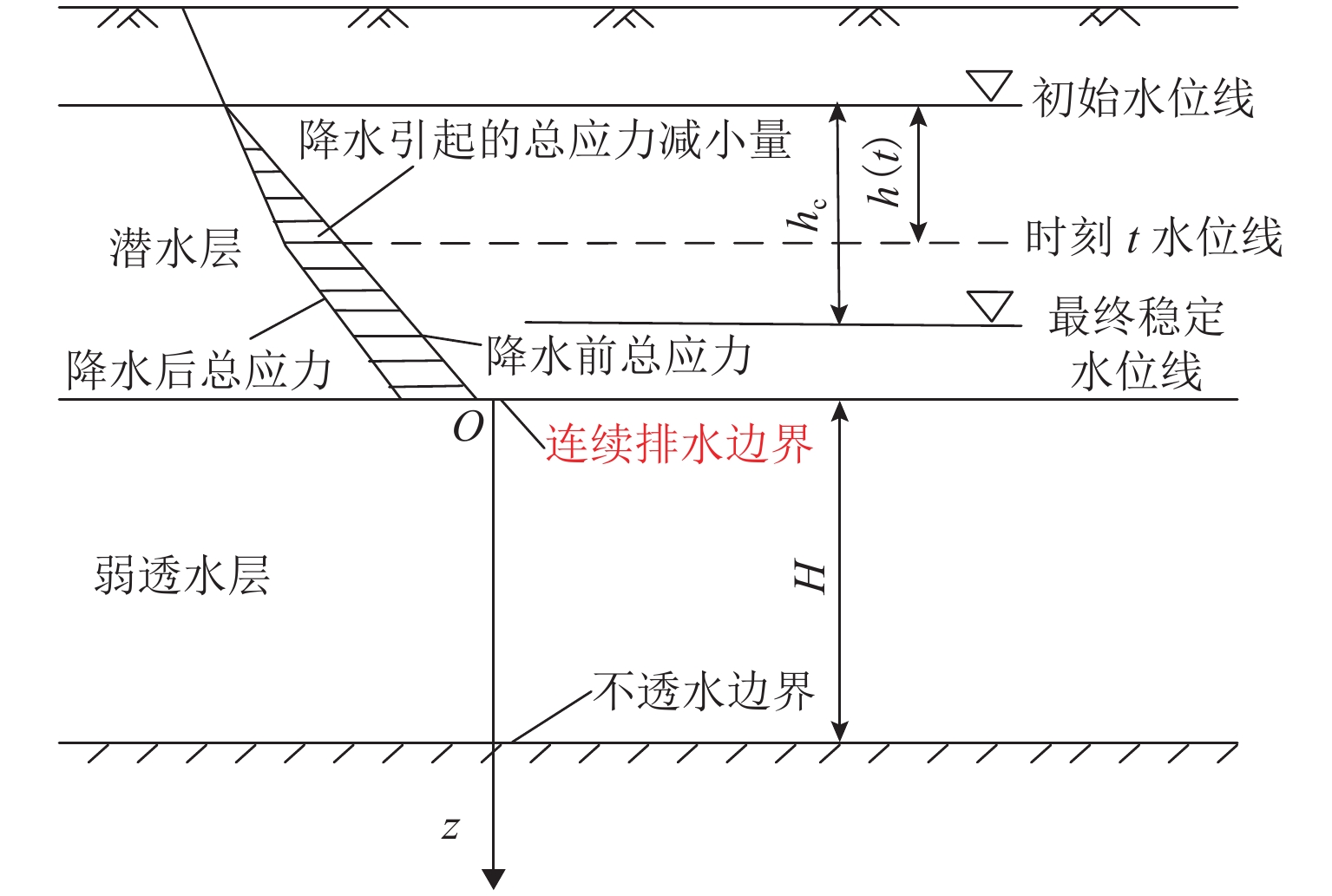
如图1所示，潜水层发生大面积抽降水，其水位随时间不断下降. 时刻t潜水层地下水位相比初始时刻的水位下降值记为h(t)，水位稳定时的下降最终值为h_c. 潜水层中发生抽降水会同时引起其下卧的弱透水层顶面处总应力和孔隙水压力减小，但由于孔压减小幅度更大，故而有效应力增大，结果造成弱透水层发生固结沉降. 弱透水层厚度为H，顶面为连续排水边界，底面为不透水边界；竖向坐标z为以弱透水层顶面为坐标原点，以重力作用方向竖直向下为正.

图 1 连续排水边界下潜水层降水引发的弱透水层一维固结示意

Figure 1. One-dimensional consolidation of aquitard caused by the dropping of groundwater table in phreatic aquifer with continuous drainage boundary

下载: 全尺寸图片幻灯片

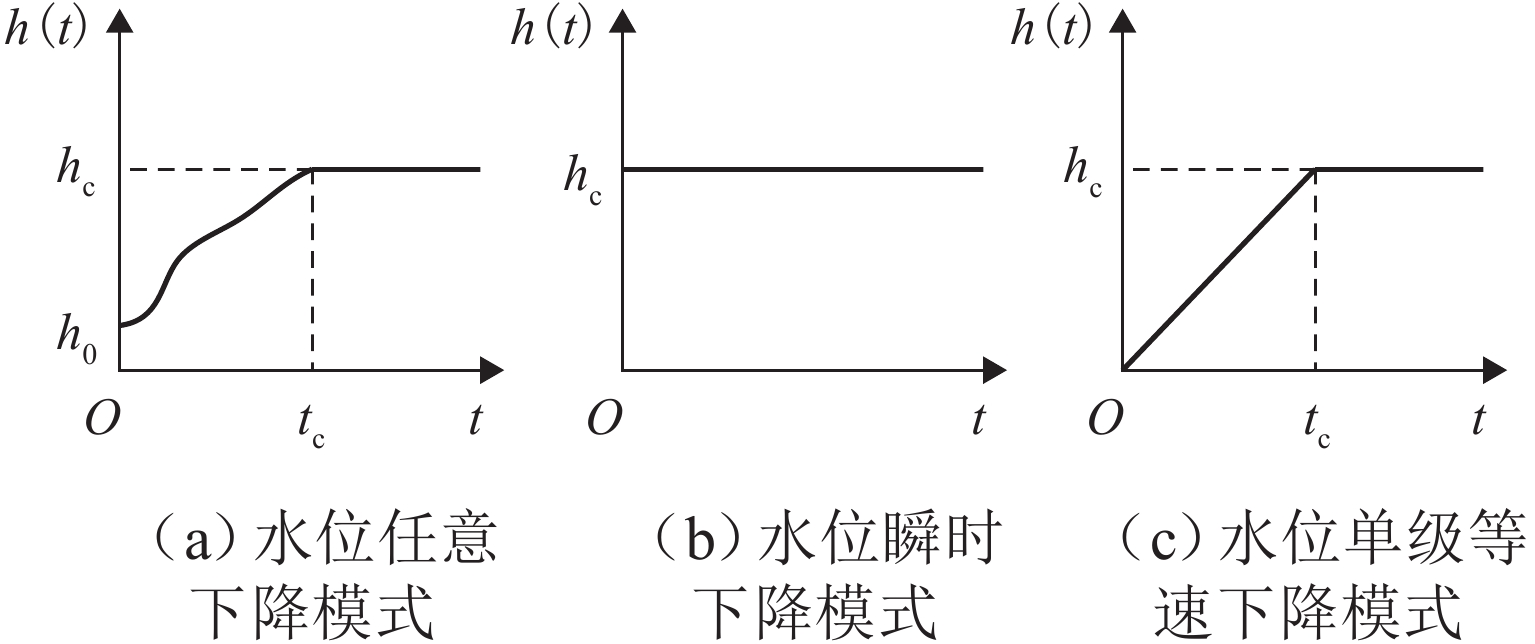
图2（a）为水位随时间任意下降模式，图2（b）和图2（c）为2种特殊降水模式下的水位下降值h(t)与t的关系. 图中：h₀为初始水位瞬时下降值，t_c为水位下降所需时间. 其中，图2（b）为水位瞬时下降模式，其数学表达式为

图 2 水位下降值随时间变化示意

Figure 2. Variation of the dropping of groundwater table with time

下载: 全尺寸图片幻灯片

$h(t){\text{ = }}{h_{\text{c}}} .$

(1)

图2（c）为水位单级等速下降模式，其对应的数学表达式为

$h(t) = \left\{ {\begin{array}{*{20}{l}} {\dfrac{{{h_{\text{c}}}}}{{{t_{\text{c}}}}}t}，\quad{t \leqslant {t_{\text{c}}}} ，\\ {{h_{\text{c}}}}，\quad{t \gt {t_{\text{c}}}} . \end{array}} \right.$

(2)

1.2 固结控制方程及求解条件

基于太沙基一维固结理论，可得潜水层水位任意下降模式下的弱透水层固结控制微分方程为

${c_{\text{v}}}\frac{{{\partial ^2}u}}{{\partial {\textit{z}^2}}} = \frac{{\partial \left( {u - \sigma } \right)}}{{\partial t}} ，$

(3)

式中：c_v为固结系数，u为超静孔隙水压力，σ为潜水层水位下降引起的总应力增量.

潜水层水位发生下降，其下降区域内的砂土重度会由饱和重度γ_sat降为天然重度γ，导致弱透水层顶面处总应力降低，可将弱透水层顶面处总应力增量σ表达为

$\sigma = (\gamma - {\gamma _{{\rm{sat}}}})h(t) .$

(4)

根据初始条件和弱透水层边界排水条件，可将其求解条件写为

$u\left( {\textit{z},0} \right) = {\sigma _0} = (\gamma - {\gamma _{{\text{sat}}}}){h_0} ,$

(5)

${\left. {\frac{{\partial u}}{{\partial \textit{z}}}} \right|_{\textit{z} = H}} = 0 ,$

(6)

式中：σ₀为初始时刻水位瞬时下降h₀引起的弱透水层顶面处总应力增量.

完全透水边界模型下，时刻t边界处超静孔压消散总量为(γ−γ_sat + γ_w)h(t)，γ_w为水的重度. 基于此，引入不改变最终消散状态的连续排水边界，将其消散过程考虑为随时间呈指数形式衰减^[14]，故弱透水层顶面处的边界条件可由式（7）表示.

$u\left( {0,t} \right) = - {\gamma _{\text{w}}}h(t) + (\gamma - {\gamma _{{\text{sat}}}}{\text{ + }}{\gamma _{\text{w}}})h(t){{\rm{e}}^{ - bt}} .$

(7)

b值越大，边界透水能力越强，可由试验模拟或者工程实测边界处孔压随时间变化曲线反演获得.

2. 固结模型的解析求解

2.1 变量代换后的控制方程及求解条件

令$\mu = u + {\gamma _{\text{w}}}h(t) - (\gamma - {\gamma _{{\text{sat}}}}{\text{ + }}{\gamma _{\text{w}}})h(t){{\rm{e}}^{ - bt}}$，$v = ( \gamma - {\gamma _{{\text{sat}}}} + {\gamma _{\text{w}}} ) h(t)(1 - {{\rm{e}}^{ - bt}})$，$ {p_{\text{c}}}{\text{ = }}{\gamma _{\text{w}}}{h_{\text{c}}} $，$ {\sigma _{\text{c}}}{\text{ = }}\left( {\gamma - {\gamma _{{\text{sat}}}}} \right){h_{\text{c}}} $，可将式（3）转化为

${c_{\text{v}}}\frac{{{\partial ^2}\mu }}{{\partial {\textit{z}^2}}} = \frac{{\partial \left( {\mu - v} \right)}}{{\partial t}} ,$

(8)

其模型的求解条件改写为

$\left\{ {\begin{array}{*{20}{l}} {\mu \left( {\textit{z},0} \right) = 0} ,\\ {{{ {\dfrac{{\partial \mu }}{{\partial \textit{z}}}} \Bigg|}_{\textit{z} = H}} = 0} ,\\ {\mu \left( {0,t} \right) = 0} . \end{array}} \right.$

(9)

2.2 超静孔隙水压力解答

根据变换后的控制方程及求解条件，可将其解的形式固定表达为

$\mu = \sum\limits_{m = 1}^\infty {\sin \left( {\frac{{M\textit{z}}}{H}} \right)} {T_{\rm{m}}}(t),$

(10)

式中：M=(2m−1)π/2，T_m(t)为关于时间t的待定函数.

将式（10）代入式（8），利用三角函数正交性化简可得

$\frac{{{c_{\text{v}}}{M^2}}}{{{H^2}}}{T_{\rm{m}}}(t){\text{ + }}{T'_{\rm{m}}} (t) = \frac{2}{M}v'(t) .$

(11)

式（11）为非齐次线性方程，运用常数变易法，结合求解条件，可求得

${T_{\rm{m}}}(t) = \frac{{\text{2}}}{M}{{\rm{e}}^{ - \tfrac{{{c_{\text{v}}}{M^{\text{2}}}}}{{{H^{\text{2}}}}}t}}\int_{\text{0}}^t {v'\left( \tau \right){{\rm{e}}^{\tfrac{{{c_{\text{v}}}{M^{\text{2}}}}}{{{H^{\text{2}}}}}\tau }}{\rm{d}}\tau } ,$

(12)

进而可得超静孔隙水压力u的普遍解为

$\begin{split} & u = \sum\limits_{m = 1}^\infty {\sin \left( {\frac{{M\textit{z}}}{H}} \right)} {T_{\rm{m}}}(t) - {\gamma _{\text{w}}}h(t) + \\ & \quad \left( {\gamma - {\gamma _{{\text{sat}}}} + {\gamma _{\text{w}}}} \right)h(t){{\rm{e}}^{ - bt}} . \end{split}$

(13)

2.3 土层平均固结度解答

根据有效应力原理，土体有效应力改变量σ_f可表达为

$\sigma _{\rm{f}} = \sigma - u .$

(14)

将式（13）代入（14），可得

$\sigma _{\rm{f}} = \left( {\gamma - {\gamma _{{\text{sat}}}} + {\gamma _{\text{w}}}} \right)h(t)\left( {1 - {{\rm{e}}^{ - bt}}} \right) - \sum\limits_{m = 1}^\infty {\sin \left( {\frac{{M\textit{z}}}{H}} \right)} {T_{\rm{m}}}(t) .$

(15)

在式（15）基础上，可得任意时刻弱透水层沉降量S(t)为

$\begin{split} & S(t) = \int_0^H {{m_{\text{v}}}\sigma_{\rm{f}} {\rm{d}}\textit{z}} = {m_{\text{v}}} \Bigg( \left( {\gamma - {\gamma _{{\text{sat}}}} + {\gamma _{\text{w}}}} \right)h(t)H\left( {1 - {{\rm{e}}^{ - bt}}} \right) - \Bigg. \\ &\quad \left.{{\sum\limits_{m = 1}^\infty {H{T_{\rm{m}}}(t)} } / M} \right) , \end{split}$

(16)

式中：m_v为体积压缩系数.

t→∞ 条件下求取极限，可得弱透水层的最终沉降量S_∞ 为

${S_\infty } = \int_0^H {{m_{\text{v}}}{{\sigma _{\rm{cf}}}}{\rm{d}}\textit{z}} = {m_{\text{v}}}\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)H ,$

(17)

式中：σ_cf为有效应力改变最终值.

其平均固结度为

$\begin{split} & U = \frac{{S(t)}}{{{S_\infty }}} = \frac{{\left( {\gamma - {\gamma _{{\text{sat}}}} + {\gamma _{\text{w}}}} \right)h(t)\left( {1 - {{\rm{e}}^{ - bt}}} \right)}}{{{\sigma _{\text{c}}} + {p_{\text{c}}}}} - \\ &\quad \sum\limits_{m = 1}^\infty {\frac{{{T_{\rm{m}}}(t)}}{{M\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}} . \end{split}$

(18)

3. 特殊降水模式下的解答

3.1 水位瞬时下降模式

根据式（1），可将水位瞬时下降模式下的总应力增量和求解条件分别对应为

$\sigma = \left( {\gamma - {\gamma _{{\text{sat}}}}} \right){h_{\text{c}}}{\text{ = }}{\sigma _{\text{c}}} ,$

(19)

$\left\{\begin{array}{l} u\left(\textit{z}\text{，}0\right)={\sigma }_{\text{c}},\\ { \dfrac{\partial u}{\partial \textit{z}}\Bigg|}_{\textit{z}=H}=0,\\ u\left(0,t\right)=-{p}_{\text{c}} + \left({\sigma }_{\text{c}} + {p}_{\text{c}}\right){{\rm{e}}}^{-bt}.\end{array}\right.$

(20)

将式（20）代入式（12），得

${T_{\rm{m}}}(t) = \frac{{2b\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)\left( {{{\rm{e}}^{{ - bt}}} - {{\rm{e}}^{{ - {\beta _{\rm{m}}}t}}}} \right)}}{{M\left( {{\beta _{\rm{m}}} - b} \right)}} ,$

(21)

式中：β_m=c_vM²/H².

该降水模式下的u为

$\begin{split} & u = \sum\limits_{m = 1}^\infty {\frac{{2B\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{{M\left( {{M^2} - B} \right)}}\sin \left( {\frac{{M\textit{z}}}{H}} \right)} \left( {{{\rm{e}}^{ - B{T_{\text{v}}}}} - {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}}} \right) - \\ & \quad {p_{\text{c}}} + \left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{ - B{T_{\text{v}}}}} , \end{split}$

(22)

式中：B=bH²/c_v；T_v为时间因子，T_v=c_vt/H².

进而获得该降水模式下时刻t弱透水层沉降量为

$\begin{split} & S(t) = {m_{\text{v}}}\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)H\left( {1 - {{\rm{e}}^{ - B{T_{\text{v}}}}}} \right) - \\ &\quad \sum\limits_{m = 1}^\infty {\frac{{2{m_{\text{v}}}B\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)H}}{{\left( {{M^2} - B} \right){M^2}}}} \left( {{{\rm{e}}^{ - B{T_{\text{v}}}}} - {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}}} \right) , \end{split}$

(23)

其对应的无量纲化平均固结度U为

$U = 1 - {{\rm{e}}^{ - B{T_{\text{v}}}}} - \sum\limits_{m = 1}^\infty {\frac{{2B}}{{\left( {{M^2} - B} \right){M^2}}}} \left( {{{\rm{e}}^{ - B{T_{\text{v}}}}} - {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}}} \right) .$

(24)

3.2 水位单级等速下降模式

由式（2）可得水位单级等速下降模式下的总应力增量和求解条件分别为

$\sigma = \left\{ {\begin{array}{*{20}{c}} {\dfrac{{{\sigma _{\text{c}}}}}{{{t_{\text{c}}}}}t}，\quad{t \leqslant {t_{\text{c}}}}， \\ {{\sigma _{\text{c}}}}，\quad{t \gt {t_{\text{c}}}} ， \end{array}} \right.$

(25)

$\left\{ {\begin{array}{*{20}{l}} {u\left( {\textit{z},0} \right) = 0} ,\\ {{{\left. {\dfrac{{\partial u}}{{\partial \textit{z}}}} \right|}_{\textit{z} = H}} = 0}, \\ {u\left( {0,t} \right) = \left\{ {\begin{array}{*{20}{l}} { - \dfrac{{{p_{\text{c}}}}}{{{t_{\text{c}}}}}t{\text{ + }}\dfrac{{\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{{{t_{\text{c}}}}}t{{\rm{e}}^{ - bt}}},\quad{t \leqslant {t_{\text{c}}}} ,\\ { - {p_{\text{c}}} + \left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{ - bt}}},\quad{t > {t_{\text{c}}}} . \end{array}} \right.} \end{array}} \right.$

(26)

同理可求得

$\qquad\quad{T_{\rm{m}}}(t)=\left\{ {\begin{array}{*{20}{l}} \dfrac{{2\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{{ - {\beta _{\rm{m}}}t}}}}}{{M{t_{\text{c}}}}}\left( \dfrac{{{{\rm{e}}^{{{\beta _{\rm{m}}}t}}} - 1}}{{{\beta _{\rm{m}}}}} - \dfrac{{{{\rm{e}}^{{({\beta _{\rm{m}}} - b)t}}} - 1}}{{{\beta _{\rm{m}}} - b}} + \dfrac{{bt{{\rm{e}}^{{({\beta _{\rm{m}}} - b)t}}}}}{{{\beta _{\rm{m}}} - b}} - \dfrac{{b{{\rm{e}}^{{({\beta _{\rm{m}}} - b)t}}} - b}}{{{{\left( {{\beta _{\rm{m}}} - b} \right)}^2}}} \right),\quad{t \leqslant {t_{\text{c}}}}, \\ \dfrac{{2\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{{ - {\beta _{\rm{m}}}t}}}}}{{M{t_{\text{c}}}}}\left( \dfrac{{{{\rm{e}}^{{{\beta _{\rm{m}}}{t_{\text{c}}}}}} - 1}}{{{\beta _{\rm{m}}}}} - \dfrac{{{{\rm{e}}^{{({\beta _{\rm{m}}} - b){t_{\text{c}}}}}} - 1}}{{{\beta _{\rm{m}}} - b}} - \dfrac{{b{{\rm{e}}^{{({\beta _{\rm{m}}} - b){t_{\text{c}}}}}} - b}}{{{{\left( {{\beta _{\rm{m}}} - b} \right)}^2}}} \right) + \dfrac{{2b\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{{ - bt}}}}}{{M\left( {{\beta _{\rm{m}}} - b} \right)}} ,\quad{t > {t_{\text{c}}}} . \end{array}} \right.$

(27)

将式（27）分别代入式（13）、（16）、（18），即可获得潜水层水位单级等速下降模式下弱透水层的超静孔压、沉降量以及固结度解析式.

3.3 超静孔压解的退化

排水边界透水性能强弱与b成正相关，b越大，其边界透水性越好. 理论上，b→＋∞ 时，弱透水层边界可视为完全透水边界，故求取此条件下超静孔压解的极限可退化为相同条件下弱透水层为完全透水边界时的孔压解.

水位瞬时下降模式下解析解退化，如式（28）. 水位单级等速下降模式下解析解退化，如式（29）.

$\qquad\qquad\qquad\begin{split} &\mathop {\lim }\limits_{b \to \infty } u = \mathop {\lim }\limits_{B \to \infty } \sum\limits_{m = 1}^\infty {\frac{{2B\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{{M\left( {{M^2} - B} \right)}}\sin \left( {\frac{{M\textit{z}}}{H}} \right)} \left( {{{\rm{e}}^{ - B{T_{\text{v}}}}} - {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}}} \right) - {p_{\text{c}}} + \left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{ - B{T_{\text{v}}}}} = \\ &\quad \sum\limits_{m = 1}^\infty {\frac{{2\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{M}\sin \left( {\frac{{M\textit{z}}}{H}} \right)} {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}} - {p_{\text{c}}} . \end{split}$

(28)

$\begin{split} &\mathop {\lim }\limits_{b \to \infty } u = \left\{ \begin{gathered} \sum\limits_{m = 1}^\infty {\sin \left( {\frac{{M\textit{z}}}{H}} \right)} \frac{{{\text{2}}\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{{M{t_{\text{c}}}}}\frac{{1 - {{\rm{e}}^{ - {\beta _{\rm{m}}}t}}}}{{{\beta _{\rm{m}}}}} - \frac{{{p_{\text{c}}}}}{{{t_{\rm{c}}}}}t= \sum\limits_{m = 1}^\infty {\frac{{{\text{2}}\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{{{M^3}{T_{{\text{vc}}}}}}\sin \left( {\frac{{M\textit{z}}}{H}} \right)} \left( {1 - {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}}} \right) - \frac{{{p_{\text{c}}}}}{{{T_{{\text{vc}}}}}}{T_{\text{v}}}, \quad t \leqslant t_{\rm{c}}, \\ \sum\limits_{m = 1}^\infty {\sin \left( {\frac{{M\textit{z}}}{H}} \right)} \frac{{{\text{2}}\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right){{\rm{e}}^{ - {\beta _{\rm{m}}}t}}}}{{M{t_{\text{c}}}}}\frac{{\left( {{{\rm{e}}^{{{\beta _{\rm{m}}}{t_{\text{c}}}}}} - 1} \right)}}{{{\beta _{\rm{m}}}}} - {p_{\text{c}}} = \sum\limits_{m = 1}^\infty {\frac{{{\text{2}}\left( {{\sigma _{\text{c}}} + {p_{\text{c}}}} \right)}}{{{M^3}{T_{{\text{vc}}}}}}\sin \left( {\frac{{M\textit{z}}}{H}} \right)} {{\rm{e}}^{ - {M^2}{T_{\text{v}}}}}\left( {{{\rm{e}}^{{{M^2}{T_{{\text{vc}}}}}}} - 1} \right) - {p_{\text{c}}},\quad t > t_{\rm{c}}, \\ \end{gathered} \right. \end{split}$

(29)

式中：T_vc为水位下降历时t_c所对应的无量纲时间因子，T_vc=c_vt_c/H².

在b→ + ∞ 时，2种特殊降水模式下的超静孔压解可以退化为文献[21]中相同模型完全透水边界条件下的相应解答，初步验证了本文解答的正确性.

4. 与室内模型试验结果对比

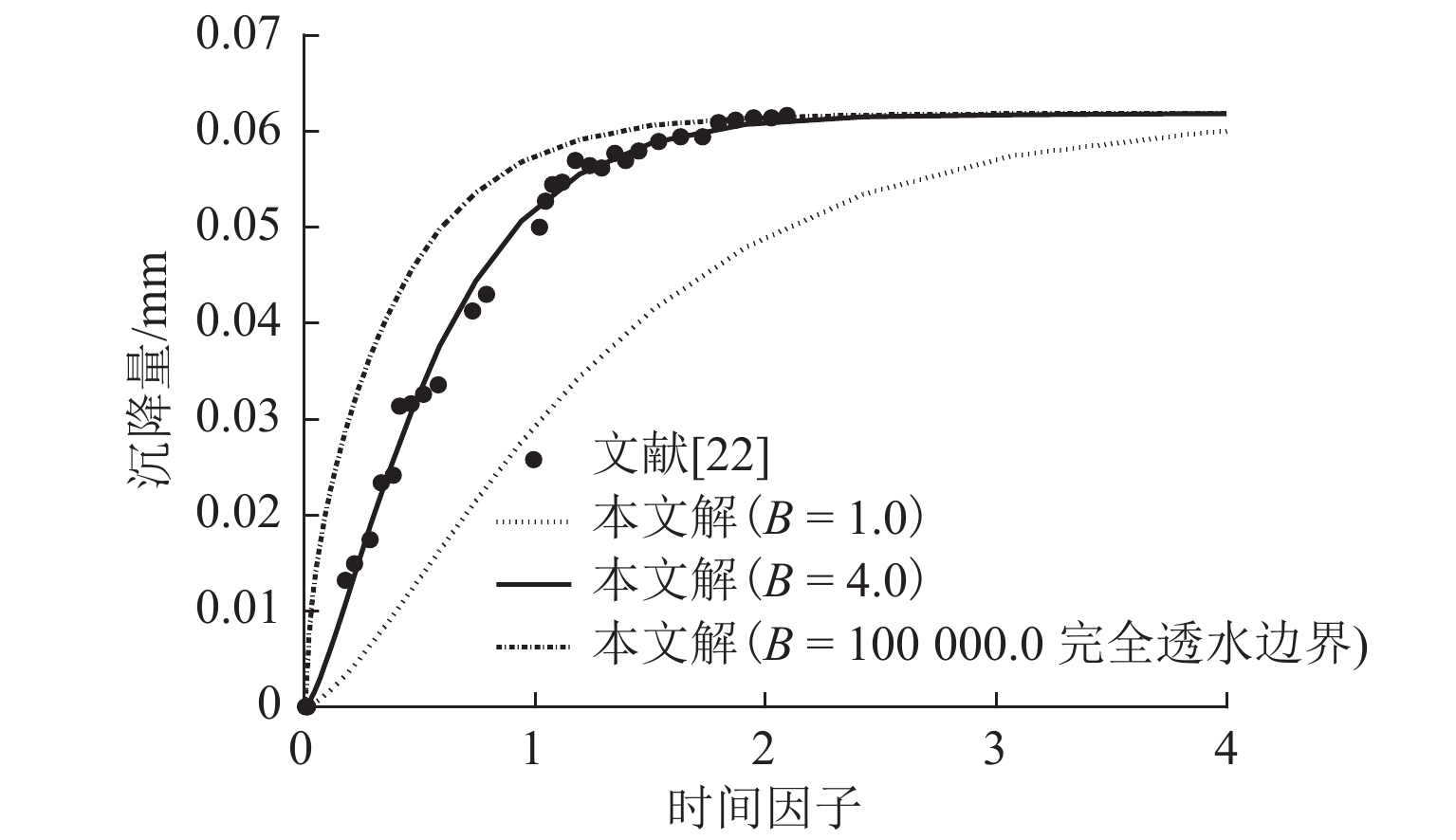
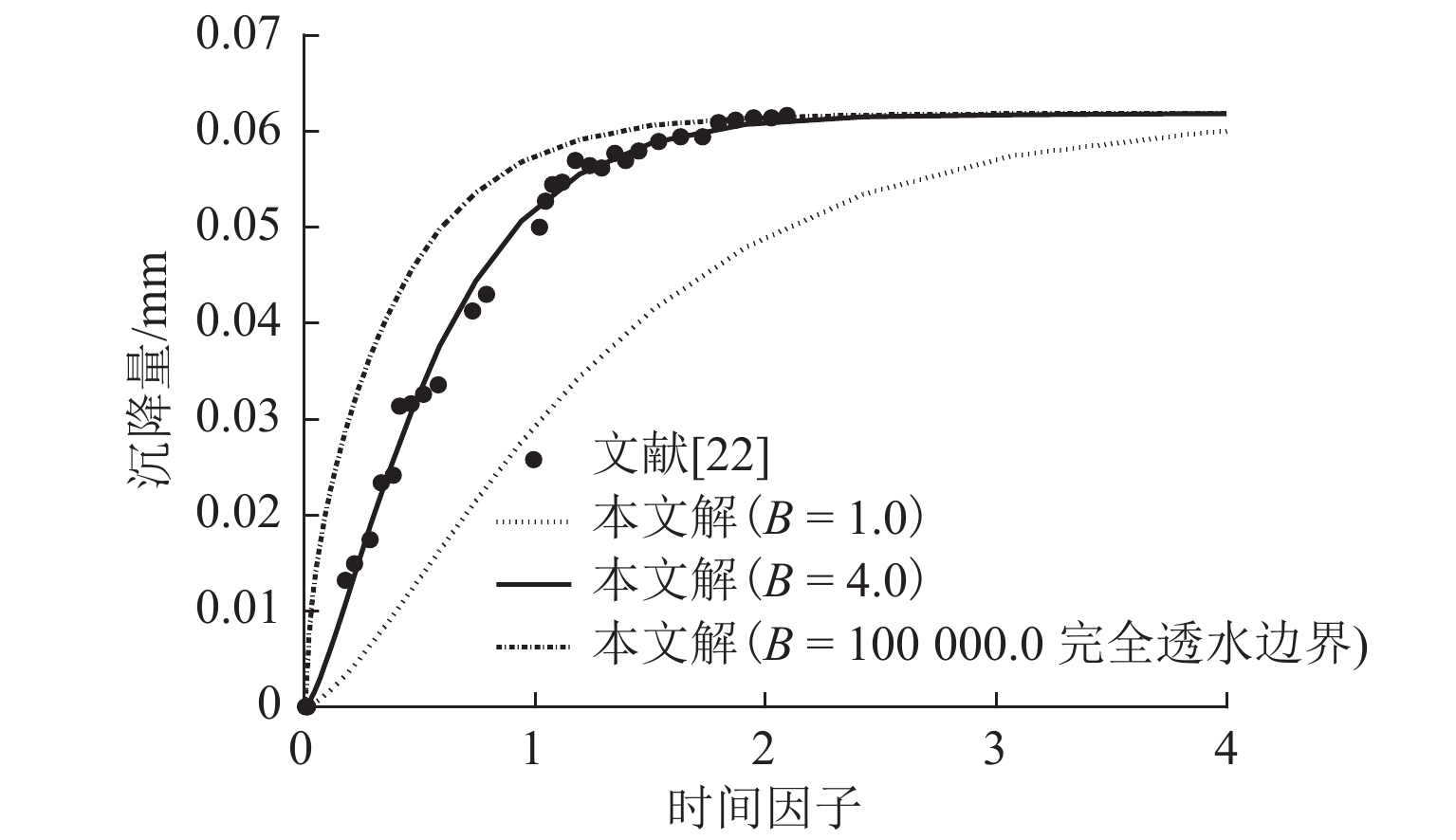
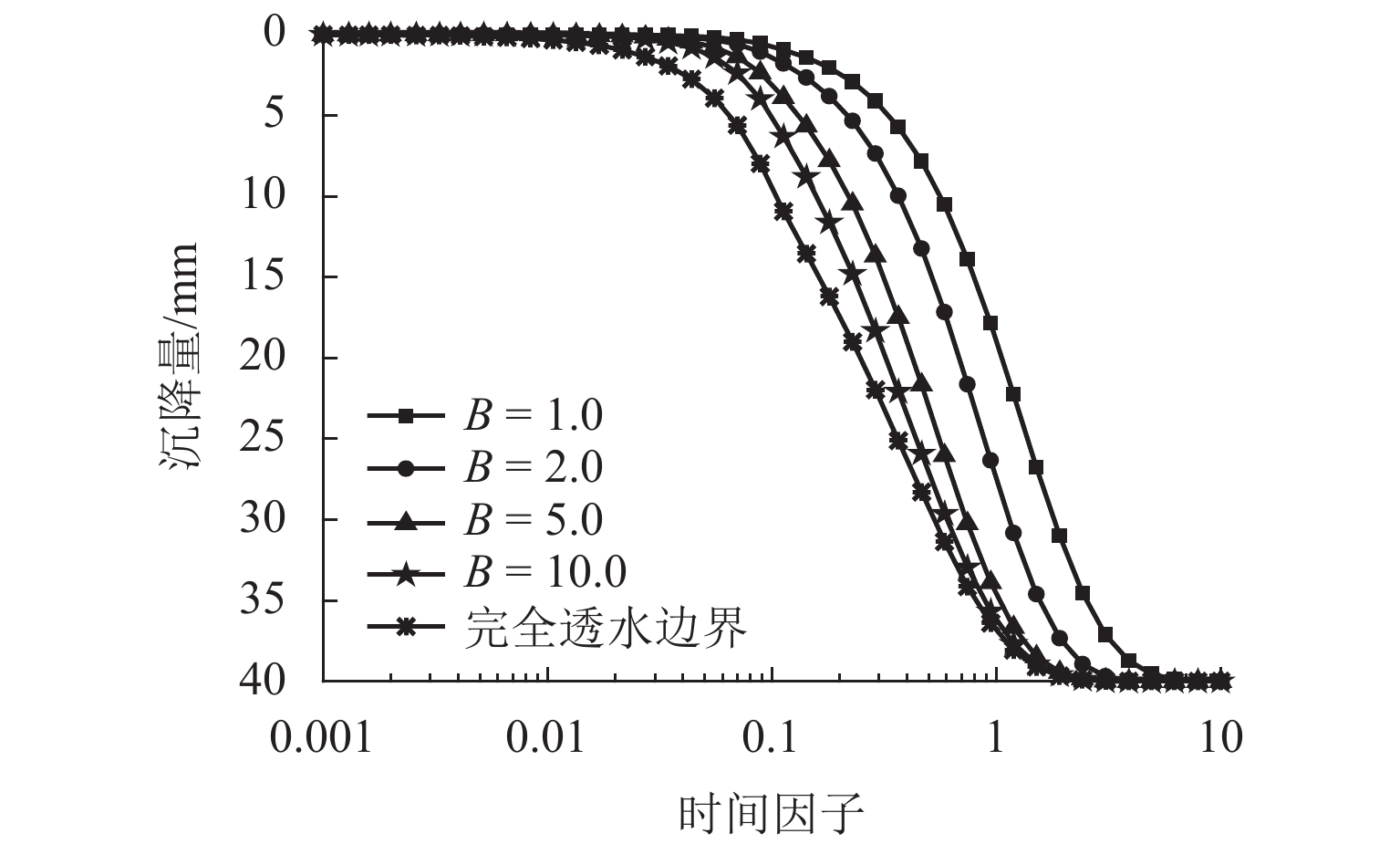
为进一步验证本文解与程序的可靠性，将不同界面参数B值下本文解答与文献[22]中的试验实测沉降曲线（图2.13）进行对比. 涉及参数：H=30 cm，t_c=60 min，h_c=20 cm，S_∞=0.0618 mm，忽略水位下降区域内砂土重度的改变，可反推得弱透水层体积压缩系数m_v=0.103 MPa⁻¹. 弱透水层渗透系数较小，其值介于10⁻¹⁰～10⁻⁹ m/s^[23]，取范围中间值5 × 10⁻¹⁰ m/s. 当取B=100000.0时，已近似等同于完全透水边界. 沉降对比结果如图3所示，可看出，利用解析获得的沉降值与实测值随时间发展趋势一致，且当B=4.0时两者的吻合度较好，误差在13%以内，再次证实计算弱透水层沉降变形时考虑连续排水边界的必要性，其计算结果与实测试验值更符合.

图 3 本文解与文献[22]试验结果的沉降对比

Figure 3. Comparison of settlement between solutions in this paper and experimental results in reference [22]

下载: 全尺寸图片幻灯片

5. 算例及参数B对固结性状的影响

界面系数b反映着排水边界透水能力的强弱，通过改变其对应的无量纲界面参数B的大小，可得到不同排水边界条件的固结模型. 以潜水层水位单级等速下降为例，基于表1中相关计算参数，利用本文解分别计算绘制在不同界面参数B值下潜水层水位单级等速下降引发的弱透水层固结沉降曲线，分析B对固结性状的影响.

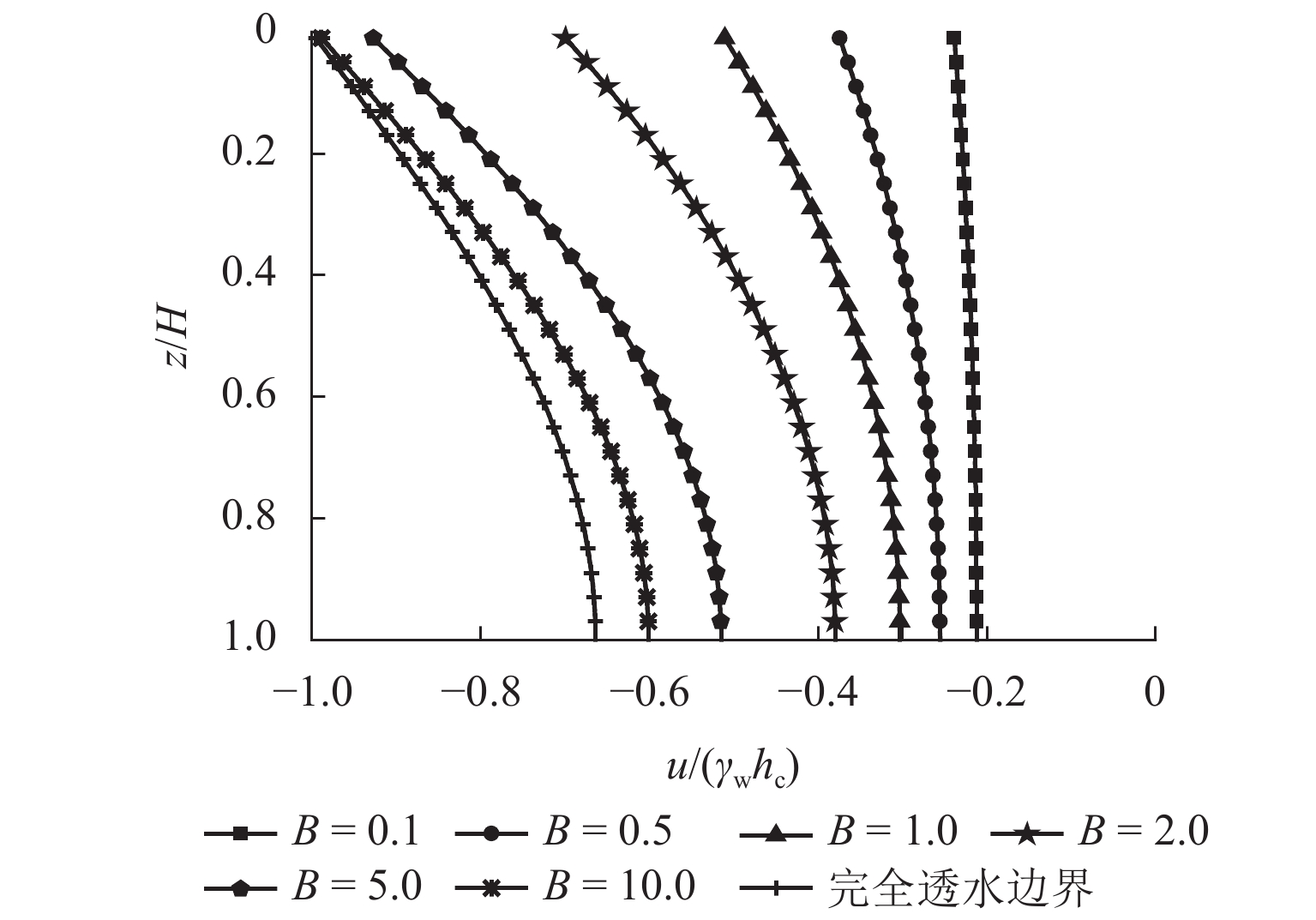
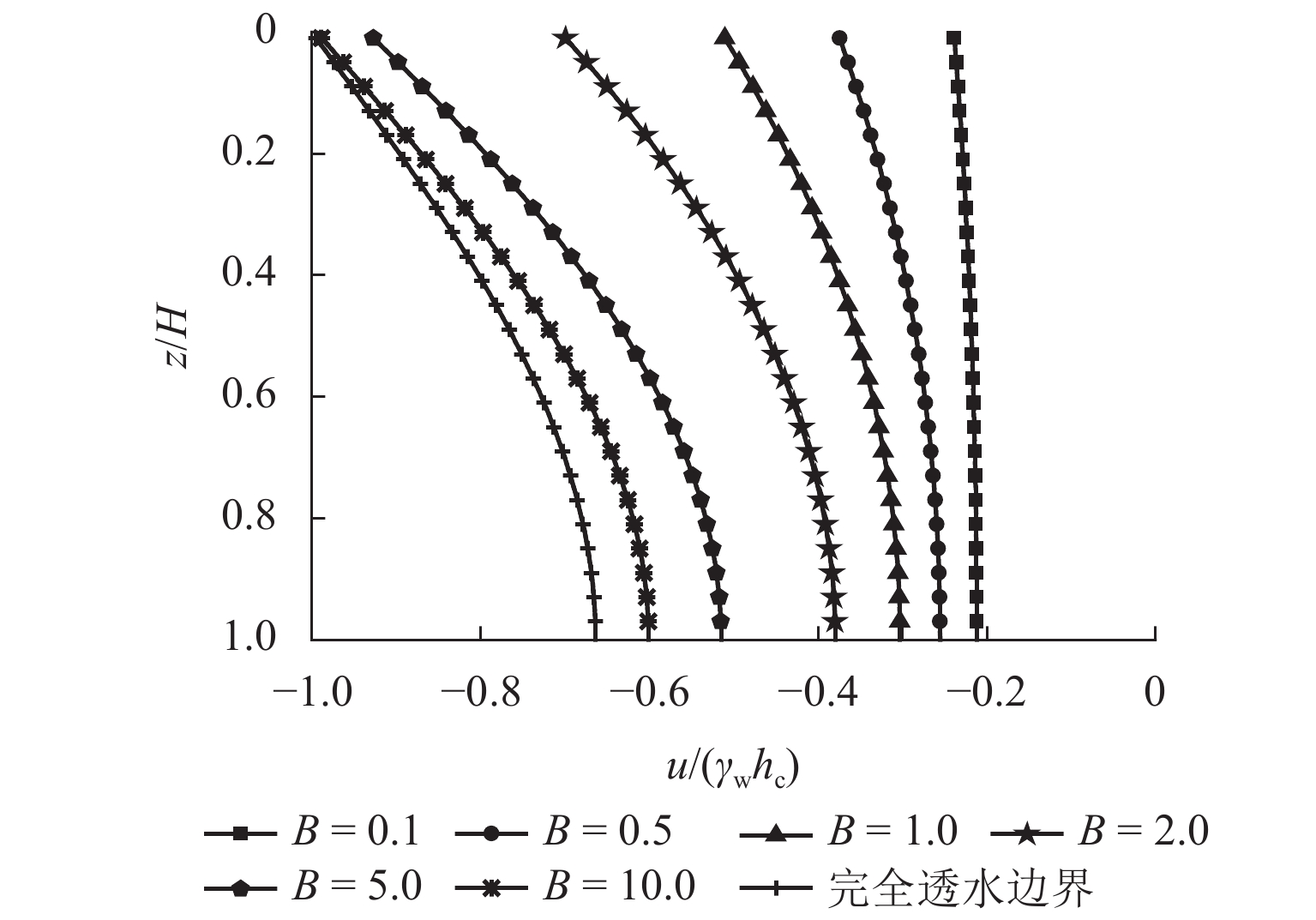
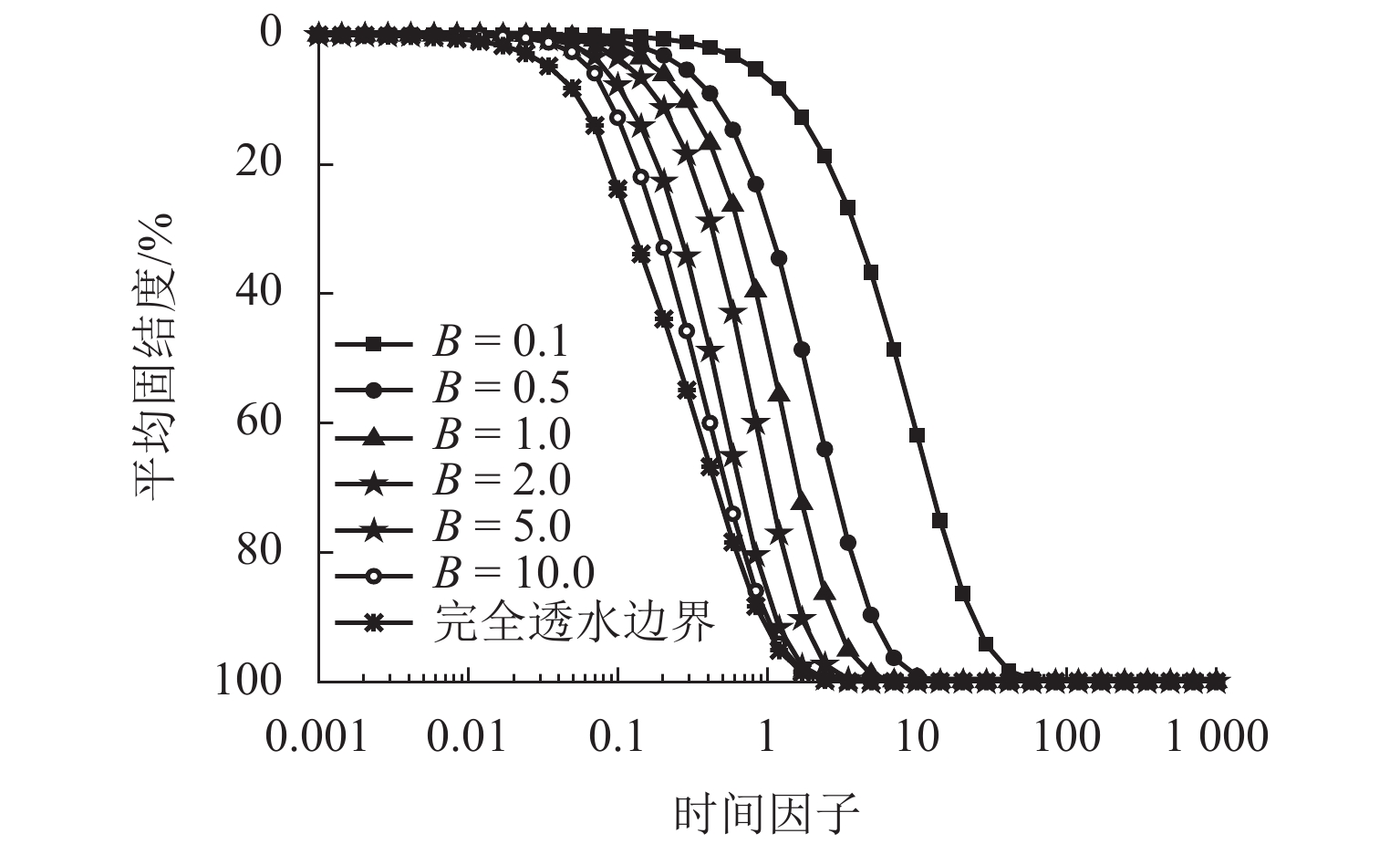
表 1 固结性状分析采用的模型参数

Table 1. Model parameters adopted in consolidation behavior analysis

参数	数值
γ_sat/（kN·m⁻³）	20
γ/（kN·m⁻³）	18
γ_w/（kN·m⁻³）	10
H/m	10
m_v/MPa⁻¹	0.1
T_vc	0.1
h_c/m	5

下载: 导出CSV

| 显示表格

图4为不同界面参数B值，T_v=0.5时超静孔压沿深度分布曲线. 从图中可看出，B越大，相同深度处的超静孔压越小，说明随着B值的增大（边界排水能力增强），其孔压消散速率越快，完全透水边界条件下土中超静孔压消散最快.

图 4 B对超静孔压沿深度分布曲线的影响

Figure 4. Influence of B on distribution curves of excess pore pressure with depth

下载: 全尺寸图片幻灯片

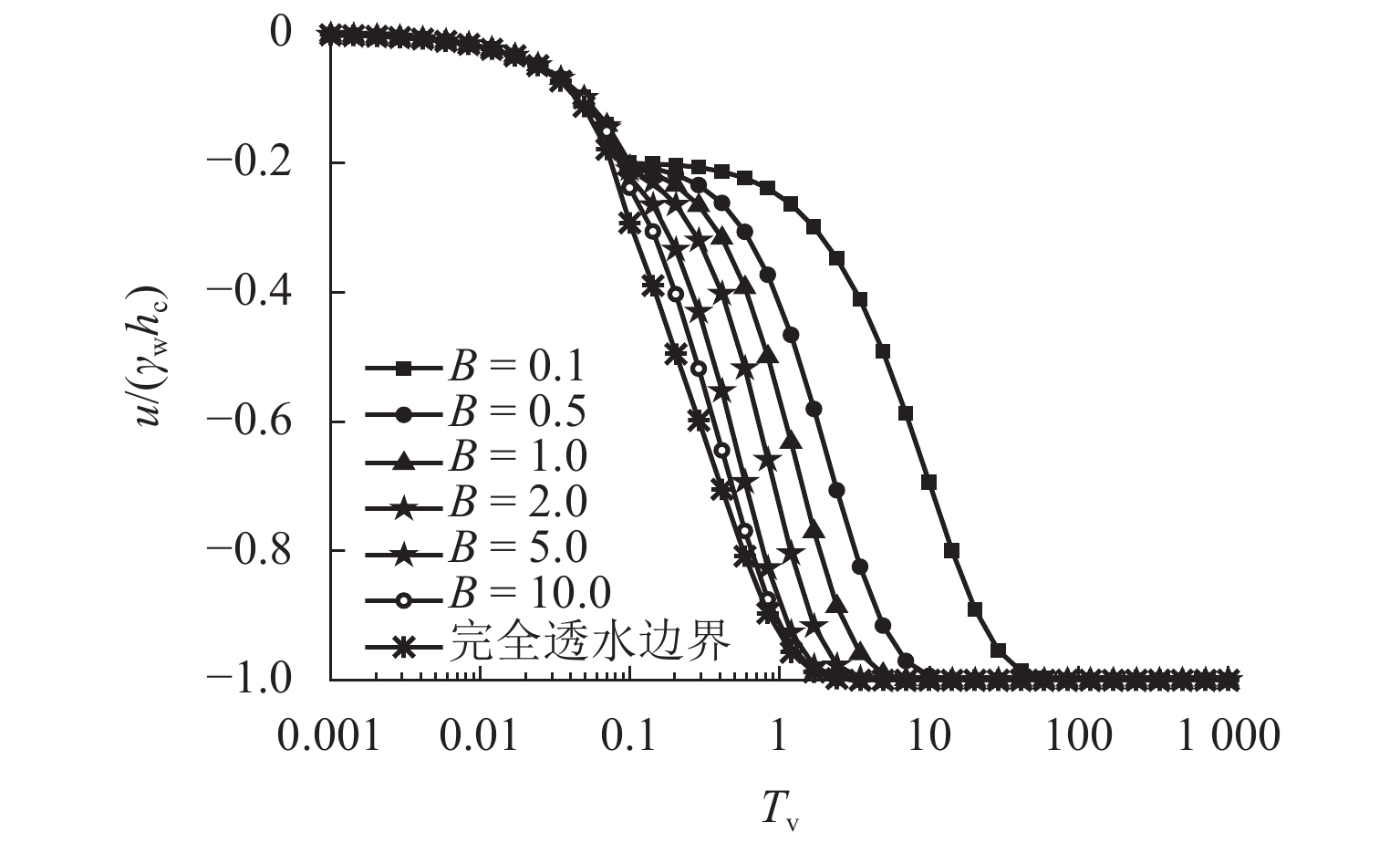
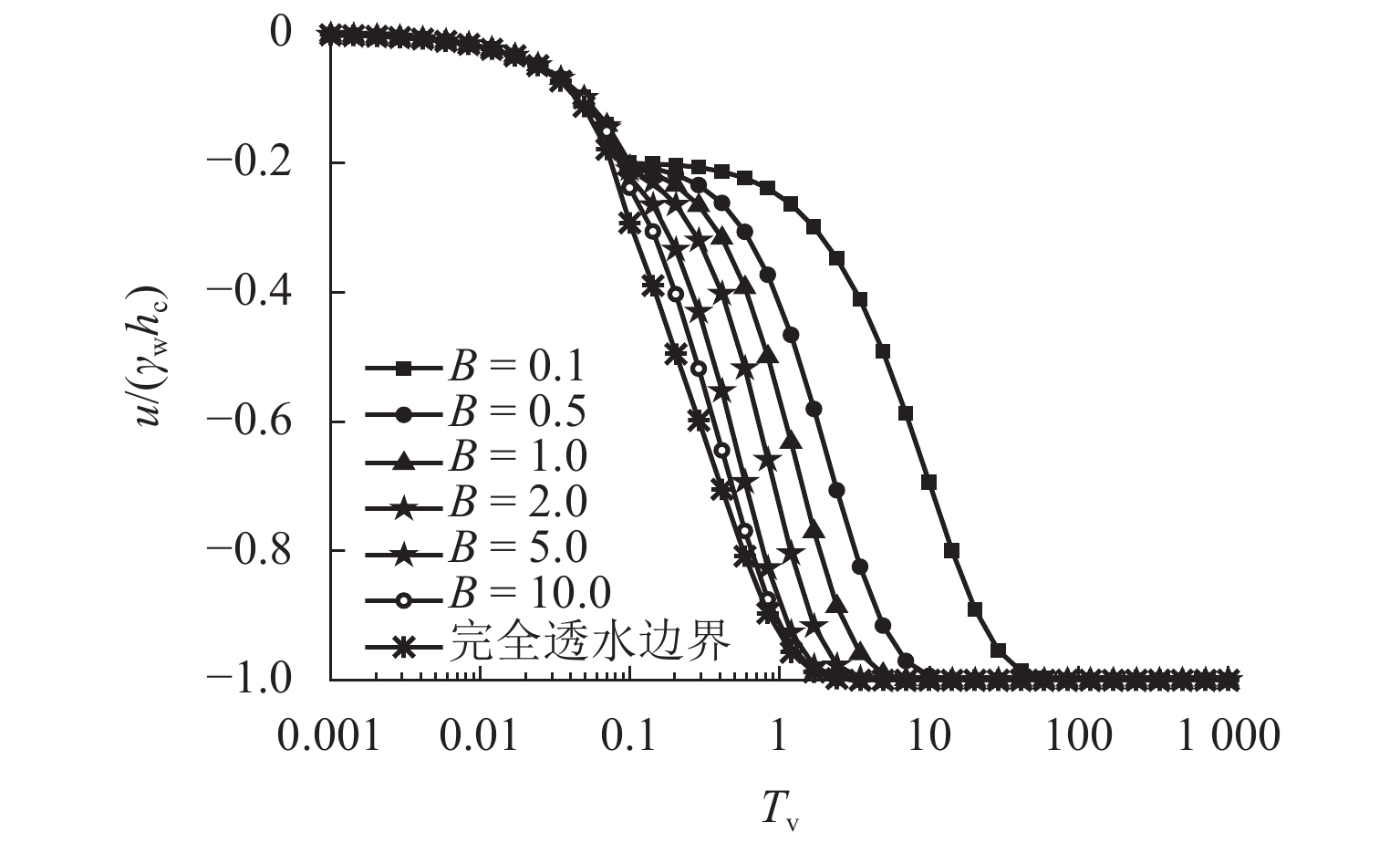
图5为不同界面参数B值，z/H=0.5深度处超静孔隙水压力随时间发展曲线. 由图可知，降水完成后，B值越大（边界透水能力越强），相同时间因子下的超静孔压越小，说明B越大，孔压的消散速率越快，其消散曲线越接近完全透水边界条件下超静孔压随时间发展曲线，进一步反映了不同透水边界情况对超静孔压消散的影响.

图 5 B对超静孔压随时间发展曲线的影响

Figure 5. Influence of B on development curves of excess pore pressure with time

下载: 全尺寸图片幻灯片

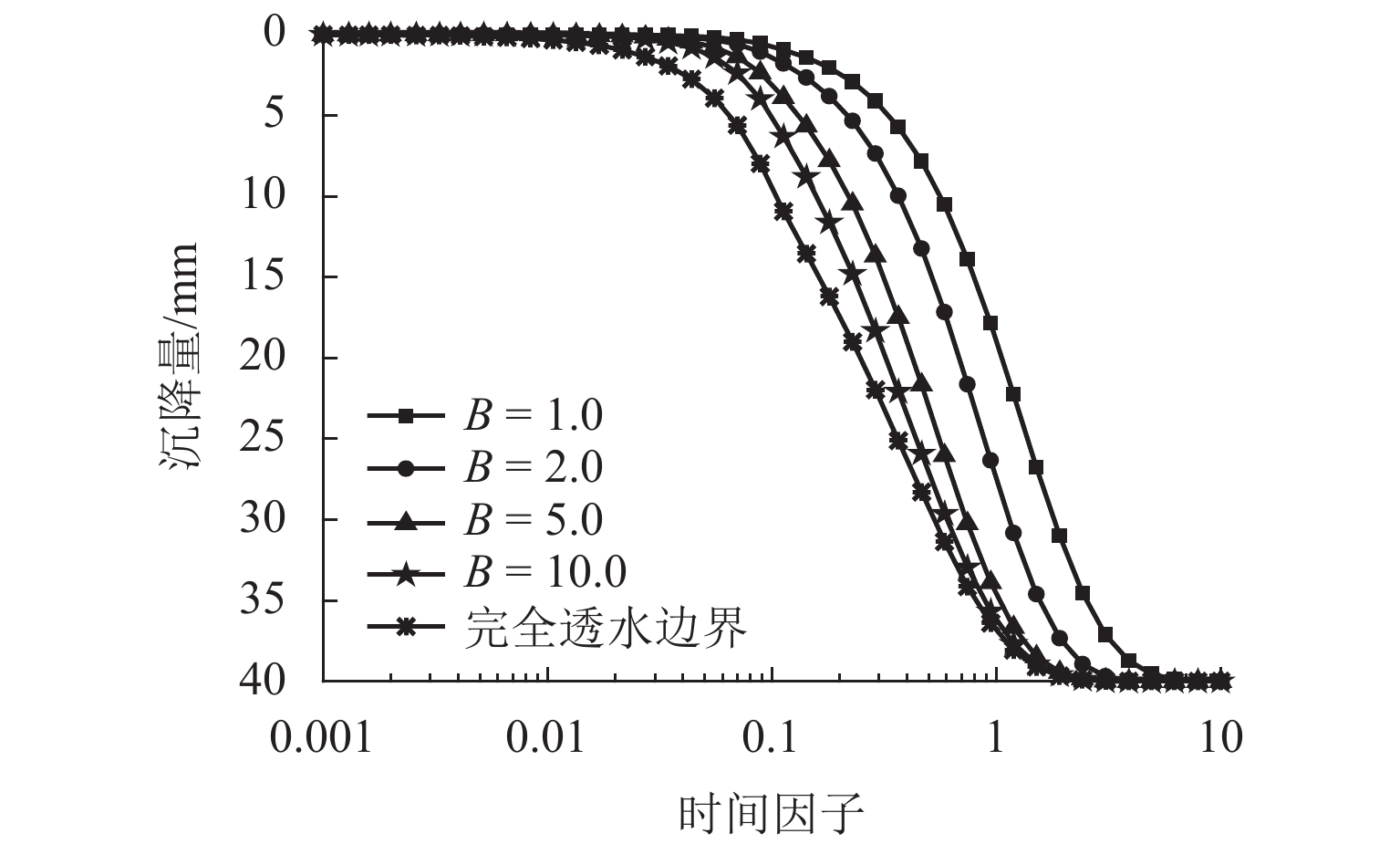
图6为不同界面参数B下弱透水层沉降量随时间发展曲线. 由图5固结性状可知，B越大，排水边界透水能力越强，相同时间因子下的孔压消散速率越快，故其沉降量越大. 界面参数B只影响排水速率，并不影响固结完成的最终状态，故B值不影响最终沉降量，该性状可由式（17）直接得出.

图 6 B对沉降量的影响

Figure 6. Influence of B on settlement

下载: 全尺寸图片幻灯片

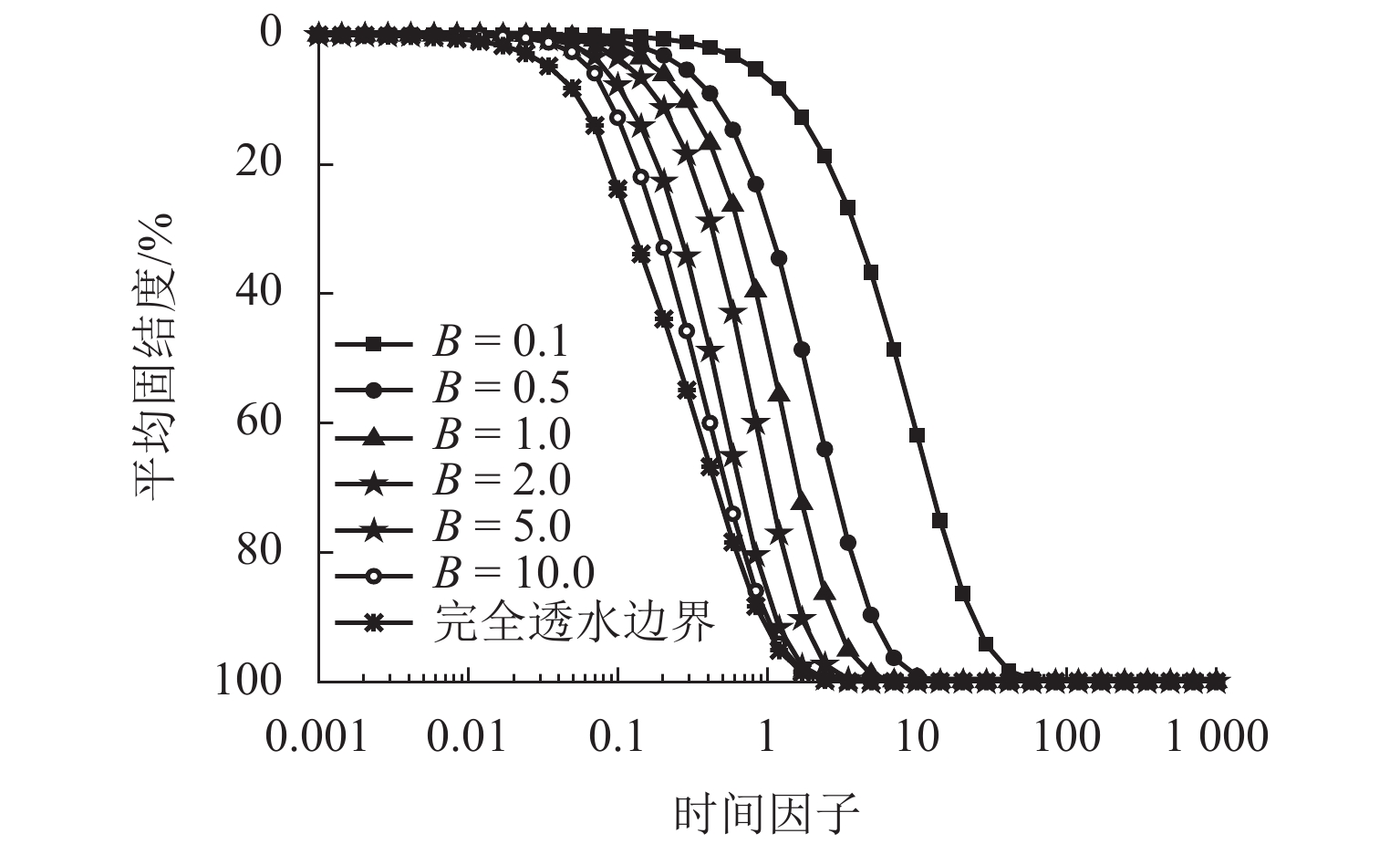
同样的固结性状体现在固结度曲线中，图7为不同B值下平均固结度随时间发展曲线. B越大，相同时间因子下超静孔压消散速率越快，最终孔压状态相同，故其相应的固结度越大，在完全透水边界条件下的固结度最大，最先完成固结.

图 7 B对固结度的影响

Figure 7. Influence of B on consolidation degree

下载: 全尺寸图片幻灯片

6. 结　论

1）界面参数B→＋∞ 时，本文解可退化为相同固结模型下弱透水层顶面为完全透水边界时的一维固结解.

2）将本文解与室内圆柱模型试验结果对比，考虑连续排水边界下的沉降计算结果与实测沉降值具有更好的拟合度.

3）界面参数B越大（透水性越好），孔压消散速率越快，固结速率越快，但不影响最终固结状态.

图 1 连续排水边界下潜水层降水引发的弱透水层一维固结示意

Figure 1. One-dimensional consolidation of aquitard caused by the dropping of groundwater table in phreatic aquifer with continuous drainage boundary

下载: 全尺寸图片幻灯片

图 2 水位下降值随时间变化示意

Figure 2. Variation of the dropping of groundwater table with time

下载: 全尺寸图片幻灯片

图 3 本文解与文献[22]试验结果的沉降对比

Figure 3. Comparison of settlement between solutions in this paper and experimental results in reference [22]

下载: 全尺寸图片幻灯片

图 4 B对超静孔压沿深度分布曲线的影响

Figure 4. Influence of B on distribution curves of excess pore pressure with depth

下载: 全尺寸图片幻灯片

图 5 B对超静孔压随时间发展曲线的影响

Figure 5. Influence of B on development curves of excess pore pressure with time

下载: 全尺寸图片幻灯片

图 6 B对沉降量的影响

Figure 6. Influence of B on settlement

下载: 全尺寸图片幻灯片

图 7 B对固结度的影响

Figure 7. Influence of B on consolidation degree

下载: 全尺寸图片幻灯片

表 1 固结性状分析采用的模型参数

Table 1. Model parameters adopted in consolidation behavior analysis

参数数值

γ_sat/（kN·m⁻³） 20
γ/（kN·m⁻³） 18
γ_w/（kN·m⁻³） 10
H/m 10
m_v/MPa⁻¹ 0.1
T_vc 0.1
h_c/m 5

下载: 导出CSV

参考文献(23)

[1]	CHEN C X, PEI S P, JIAO J. Land subsidence caused by groundwater exploitation in Suzhou City, China[J]. Hydrogeology Journal, 2003, 11(2): 275-287. doi: 10.1007/s10040-002-0225-5
[2]	YI L X, WANG J E, SHAO C Q, et al. Land subsidence disaster survey and its economic loss assessment in Tianjin, China[J]. Natural Hazards Review, 2010, 11(1): 35-41. doi: 10.1061/(ASCE)1527-6988(2010)11:1(35)
[3]	殷跃平,张作辰,张开军. 我国地面沉降现状及防治对策研究[J]. 中国地质灾害与防治学报,2005,16(2): 1-8. doi: 10.3969/j.issn.1003-8035.2005.02.001 YIN Yueping, ZHANG Zuochen, ZHANG Kaijun. Land subsidence and countermeasures for its prevention in China[J]. The Chinese Journal of Geological Hazard and Control, 2005, 16(2): 1-8. doi: 10.3969/j.issn.1003-8035.2005.02.001
[4]	张云,薛禹群. 抽水地面沉降数学模型的研究现状与展望[J]. 中国地质灾害与防治学报,2002,13(2): 1-6,24. ZHANG Yun, XUE Yuqun. Present sitution and prospect on the mathematical model of land subsidence due to pumping[J]. The Chinese Journal of Geological Hazard and Control, 2002, 13(2): 1-6,24.
[5]	骆冠勇,潘泓,曹洪,等. 承压水减压引起的沉降分析[J]. 岩土力学,2004,25(增2): 196-200. doi: 10.3969/j.issn.1000-7598.2004.z2.040 LUO Guanyong, PAN Hong, CAO Hong, et al. Analysis of settlements caused by decompression of confined water[J]. Rock and Soil Mechanics, 2004, 25(S2): 196-200. doi: 10.3969/j.issn.1000-7598.2004.z2.040
[6]	谢康和,陶立为,王玉林,等. 越流系统中弱透水层的一维固结解及分析[J]. 沈阳工业大学学报,2012,34(5): 581-585. XIE Kanghe, TAO Liwei, WANG Yulin, et al. One-dimensional consolidation solution and analysis for aquitard in leakage system[J]. Journal of Shenyang University of Technology, 2012, 34(5): 581-585.
[7]	黄大中. 水位变化引发的土层耦合固结变形理论研究[D]. 杭州: 浙江大学, 2014.
[8]	谢海澜,武强,赵增敏,等. 考虑非达西流的弱透水层固结计算[J]. 岩土力学,2007,28(5): 1061-1065. XIE Hailan, WU Qiang, ZHAO Zengmin, et al. Consolidation computation of aquitard considering non-Darcy flow[J]. Rock and Soil Mechanics, 2007, 28(5): 1061-1065.
[9]	张玮鹏,谢康和,吕文晓,等. 抽水引起的有起始比降饱和土固结解析解[J]. 中南大学学报(自然科学版),2016,47(3): 875-881. ZHANG Weipeng, XIE Kanghe, LÜ Wenxiao, et al. Analytical solution to one-dimensional consolidation of saturated soil with threshold gradient induced by groundwater pumping[J]. Journal of Central South University (Science and Technology), 2016, 47(3): 875-881.
[10]	晏凌晗. 水位下降引发的软土层一维流变固结解析与试验研究[D]. 杭州: 浙江大学, 2017.
[11]	吴浩,谢康和,黄大中. 第二类越流系统中结构性弱透水土层一维固结解析解[J]. 岩土工程学报,2014,36(9): 1688-1695. WU Hao, XIE Kanghe, HUANG Dazhong. Analytical solution for one-dimensional consolidation of structured aquitard soils in second kind of leakage system[J]. Chinese Journal of Geotechnical Engineering, 2014, 36(9): 1688-1695.
[12]	夏长青. 成层结构性软土非线性大应变流变固结理论研究[D]. 杭州: 浙江大学, 2018.
[13]	李传勋,江留慧. 抽降水引发的弱透水层非线性固结解析解[J]. 工程科学与技术,2021,53(3): 106-114. LI Chuanxun, JIANG Liuhui. Analytical solutions for nonlinear consolidation of aquitard induced by the dropping of groundwater table[J]. Advanced Engineering Sciences, 2021, 53(3): 106-114.
[14]	梅国雄,夏君,梅岭. 基于不对称连续排水边界的太沙基一维固结方程及其解答[J]. 岩土工程学报,2011,33(1): 28-31. MEI Guoxiong, XIA Jun, MEI Ling. Terzaghi’s one-dimensional consolidation equation and its solution based on asymmetric continuous drainage boundary[J]. Chinese Journal of Geotechnical Engineering, 2011, 33(1): 28-31.
[15]	冯健雪,陈征,李勇义,等. 连续排水边界条件下线性加载地基一维固结解析解[J]. 工程力学,2019,36(6): 219-226. FENG Jianxue, CHEN Zheng, LI Yongyi, et al. Analytical solution for one-dimensional consolidation of soft clayey soil with a continuous drainage boundary under linear loading[J]. Engineering Mechanics, 2019, 36(6): 219-226.
[16]	宗梦繁,吴文兵,梅国雄,等. 连续排水边界条件下土体一维非线性固结解析解[J]. 岩石力学与工程学报,2018,37(12): 2829-2838. ZONG Mengfan, WU Wenbing, MEI Guoxiong, et al. An analytical solution for one-dimensional nonlinear consolidation of soils with continuous drainage boundary[J]. Chinese Journal of Rock Mechanics and Engineering, 2018, 37(12): 2829-2838.
[17]	宗梦繁,吴文兵,梅国雄,等. 连续排水边界条件下土体一维流变固结解析解[J]. 工程力学,2019,36(9): 79-88. ZONG Mengfan, WU Wenbing, MEI Guoxiong, et al. Analytical solution for one-dimensional rheological consolidation of soil based on continuous drainage boundary[J]. Engineering Mechanics, 2019, 36(9): 79-88.
[18]	陈余,李传勋. 连续排水边界下考虑起始坡降的软黏土固结解[J]. 工程力学,2021,38(9): 161-170. CHEN Yu, LI Chuanxun. Consolidation analysis of soft clay considering the threshold hydraulic gradient and a continuous drainage boundary[J]. Engineering Mechanics, 2021, 38(9): 161-170.
[19]	张云鹏,王宗琴,宗梦繁,等. 连续排水边界下成层陆域吹填地基一维固结解析解[J]. 地质科技通报,2023,42(3): 38-45. doi: 10.19509/j.cnki.dzkq.2022.0171 ZHANG Yunpeng, WANG Zongqin, ZONG Mengfan. et al. Closed-form solution for one-dimensional consolidation analysis in reclamation area based on continuous boundary conditions[J]. Bulletin of Geological Science and Technology, 2023, 42(3): 38-45. doi: 10.19509/j.cnki.dzkq.2022.0171
[20]	苗青,闻敏杰,宗梦繁,等. 连续排水边界下非线性饱和土体一维热固结解析解[J]. 哈尔滨工业大学学报,2023,55(2): 143-150. MIAO Qing, WEN Minjie, ZONG Mengfan, et al. Analytical solution for one-dimensional thermal consolidation of nonlinear saturated soils based on continuous drainage boundary[J]. Journal of Harbin Institute of Technology, 2023, 55(2): 143-150.
[21]	江留慧. 抽降水下既有建筑物基桩承载力性状分析[D]. 镇江: 江苏大学, 2020.
[22]	顾迪. 地下水位变化条件下黏土层变形的宏微观试验研究[D]. 南京: 南京大学, 2020.
[23]	张婧玮,梁杏,葛勤,等. 江汉平原第四系弱透水层渗透系数求算方法[J]. 地球科学,2017,42(5): 761-770. ZHANG Jingwei, LIANG Xing, GE Qin, et al. Calculation method about hydraulic conductivity of quaternary aquitard in Jianghan plain[J]. Earth Science, 2017, 42(5): 761-770.