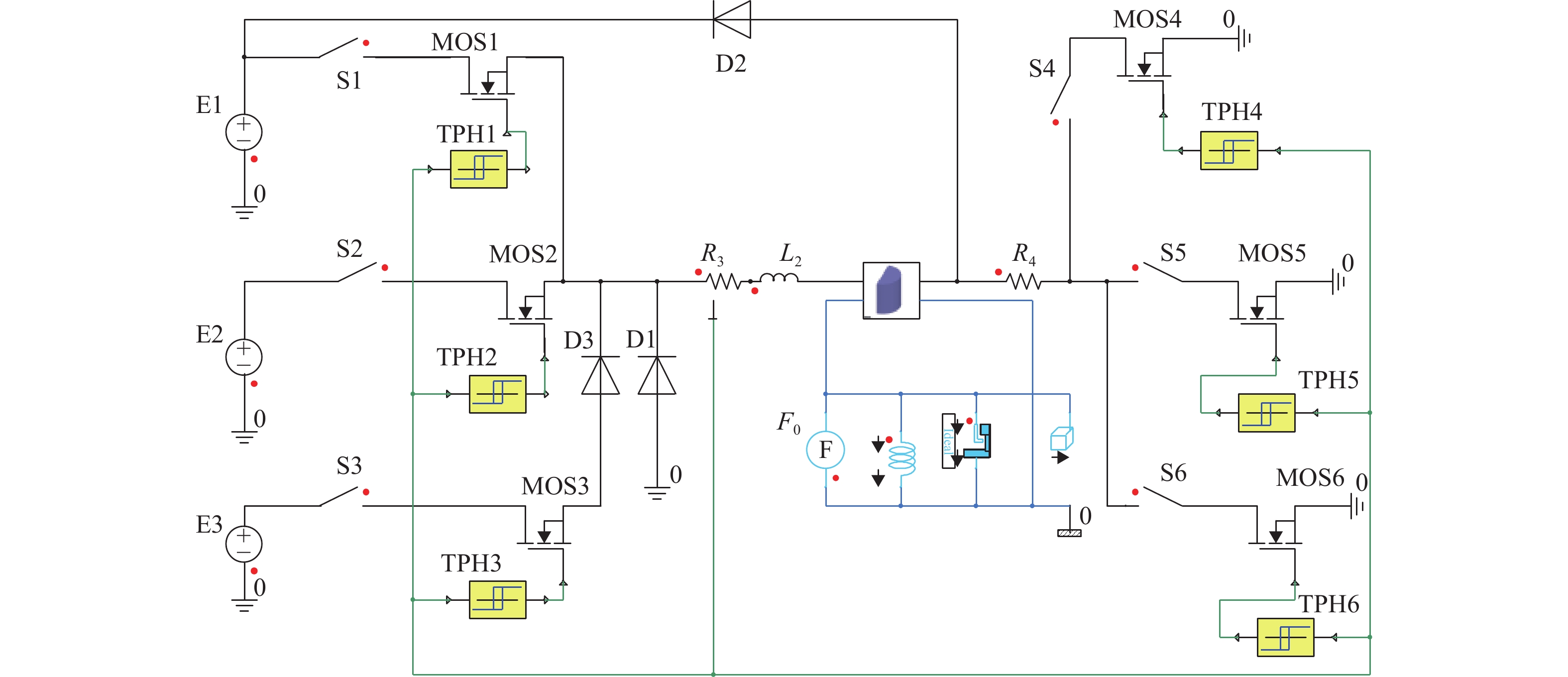
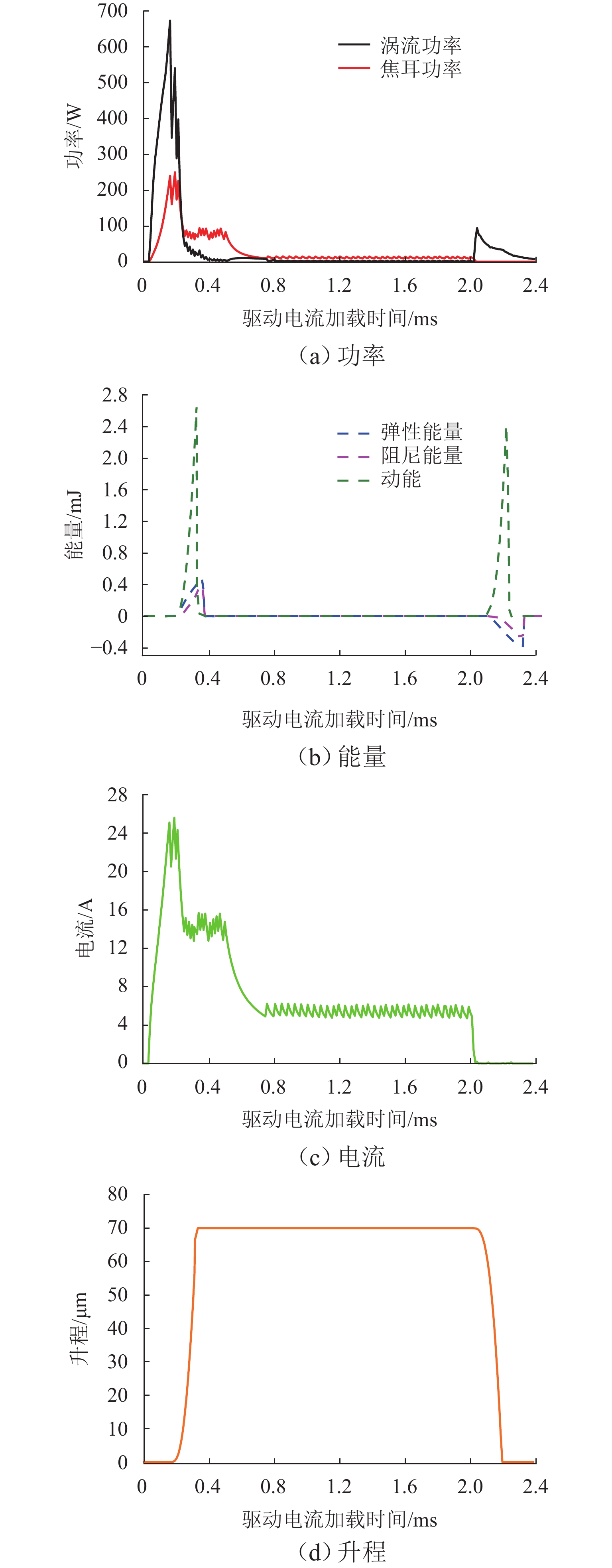
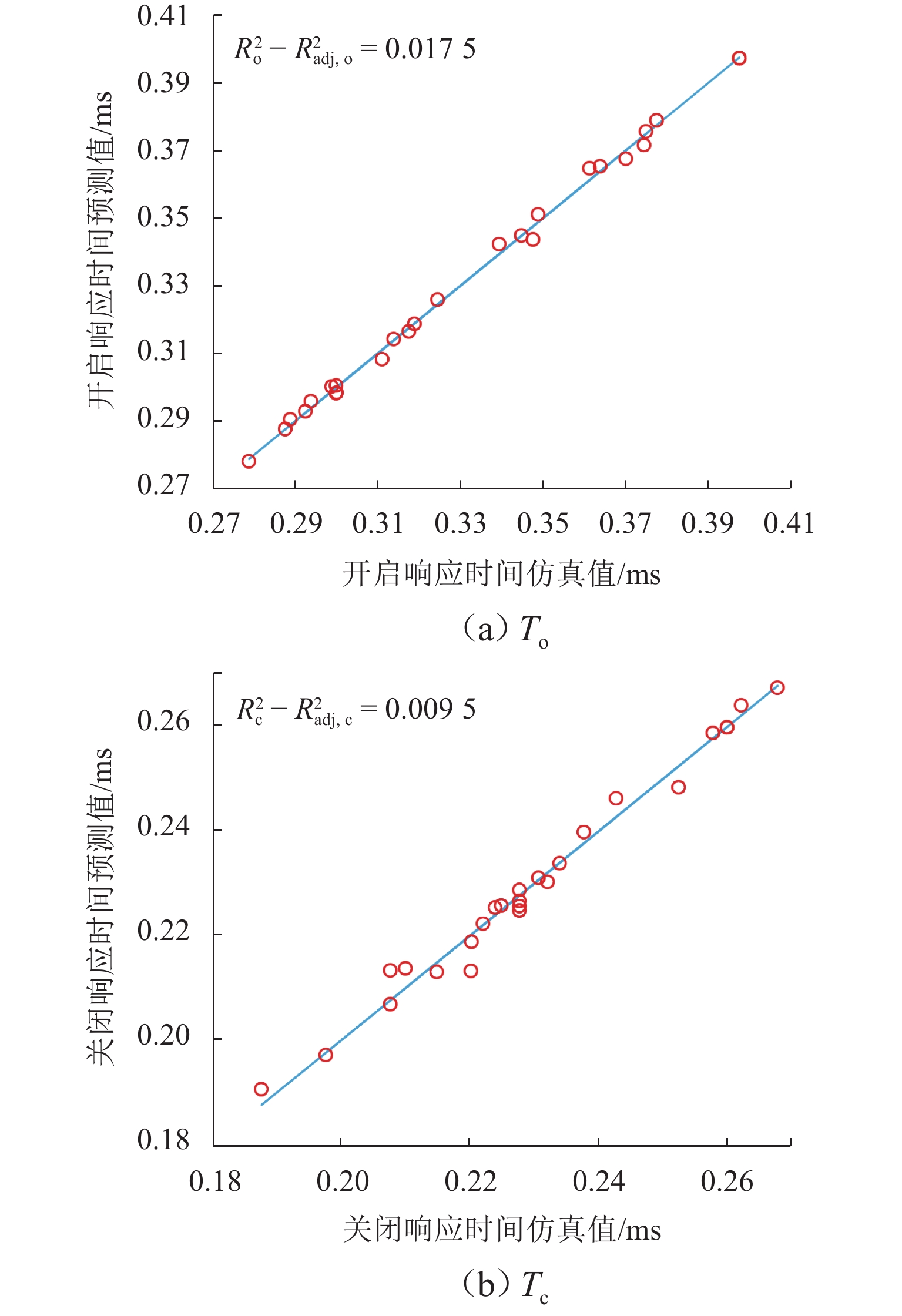
Simulation Research on Coupling Relationship Between Energy Distribution and Dynamic Response of High-Speed Solenoid Valves
-
摘要:
为研究高速电磁阀内部的能量分布及能量参数与动态响应之间的耦合关系,对高速电磁阀性能进行优化. 首先,基于有限元法建立高速电磁阀动态模型,并通过实验数据验证模型的准确性;其次,利用D最优实验设计方法和最小二乘法,构建以能量参数为因素的电磁阀动态响应特性响应面预测模型;最后,使用元效应分析法开展了高速电磁阀动态响应显著能量参数及参数交互作用的仿真分析. 研究结果表明:单参数中涡流能量
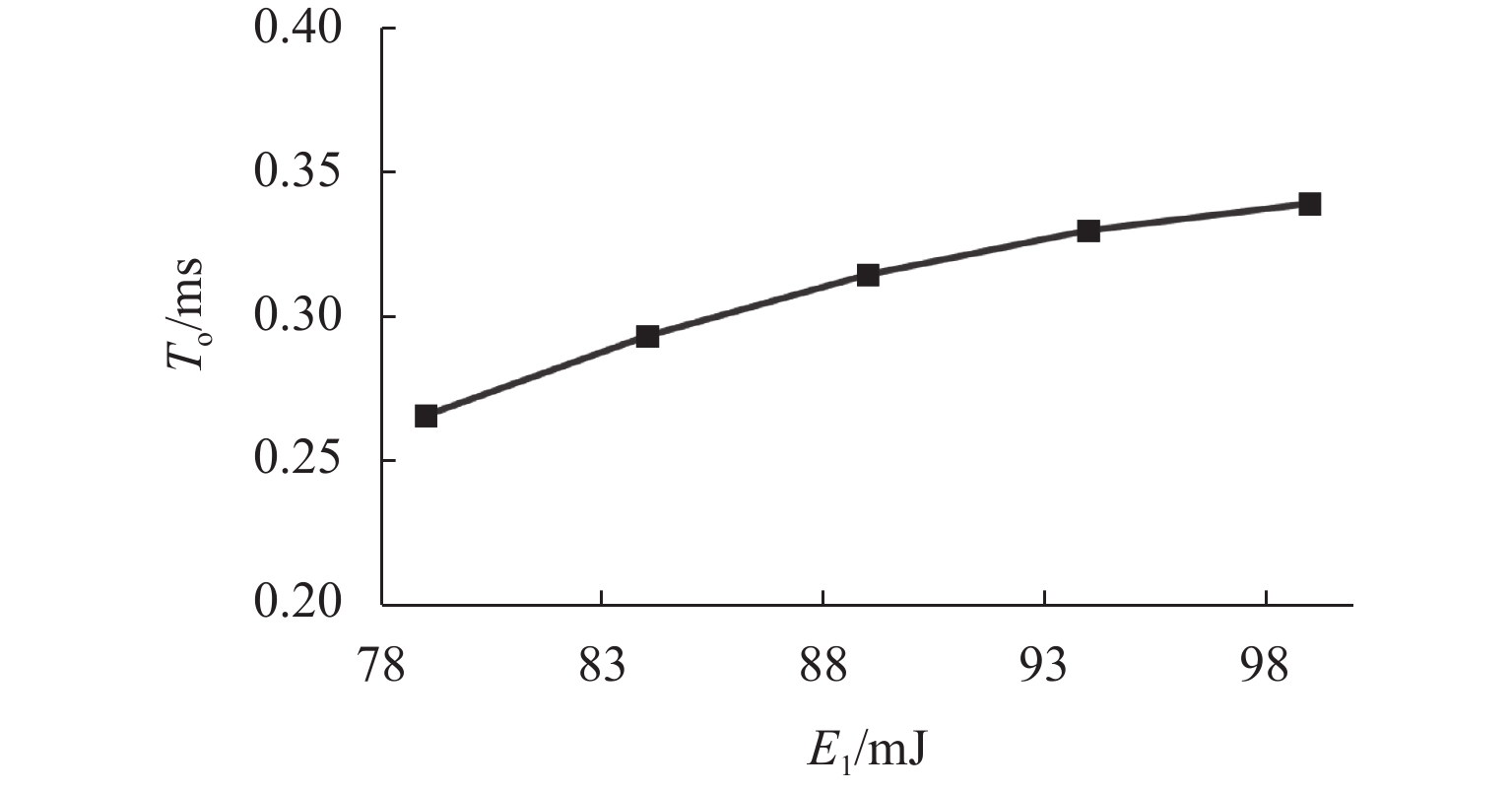
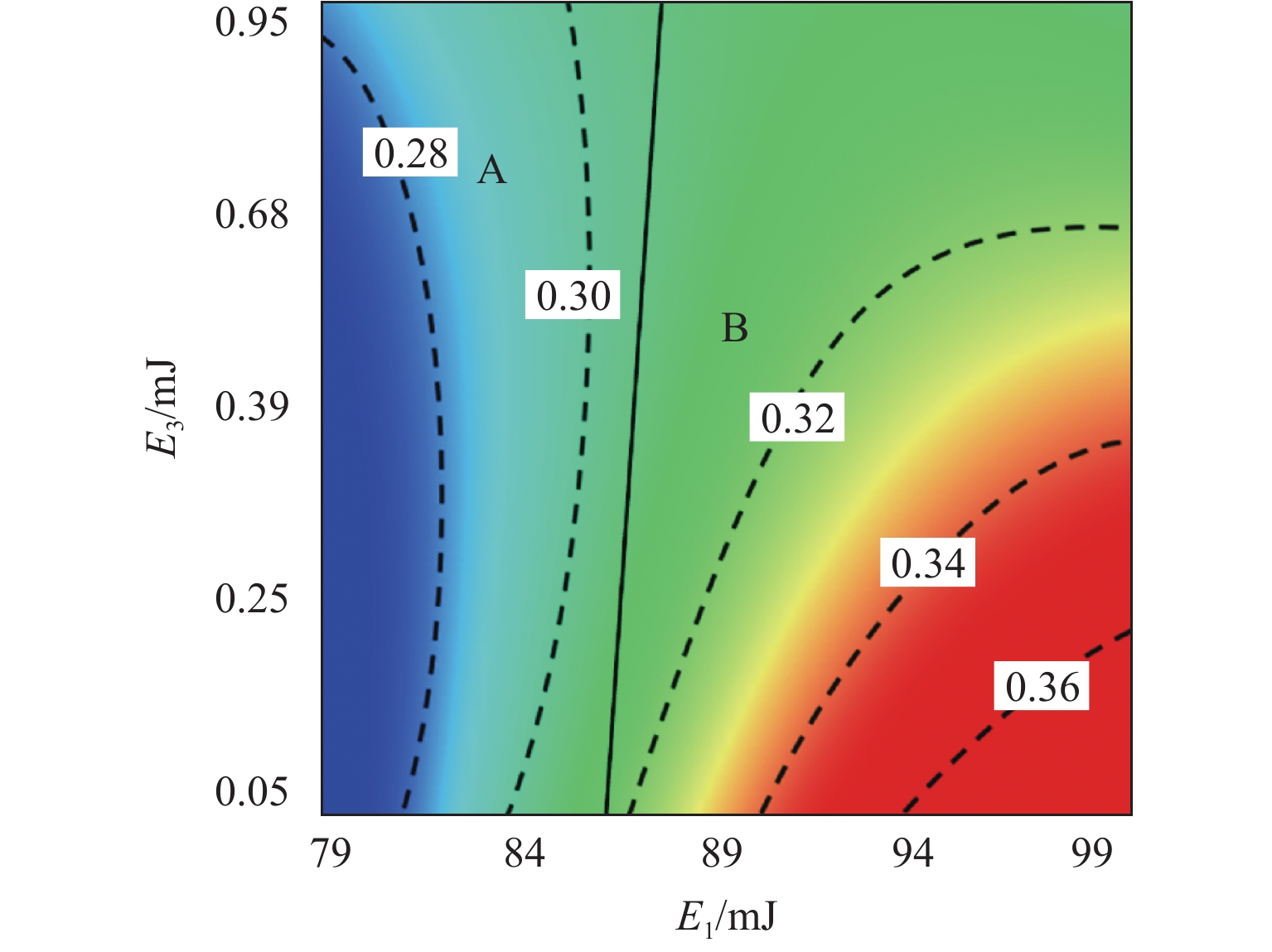
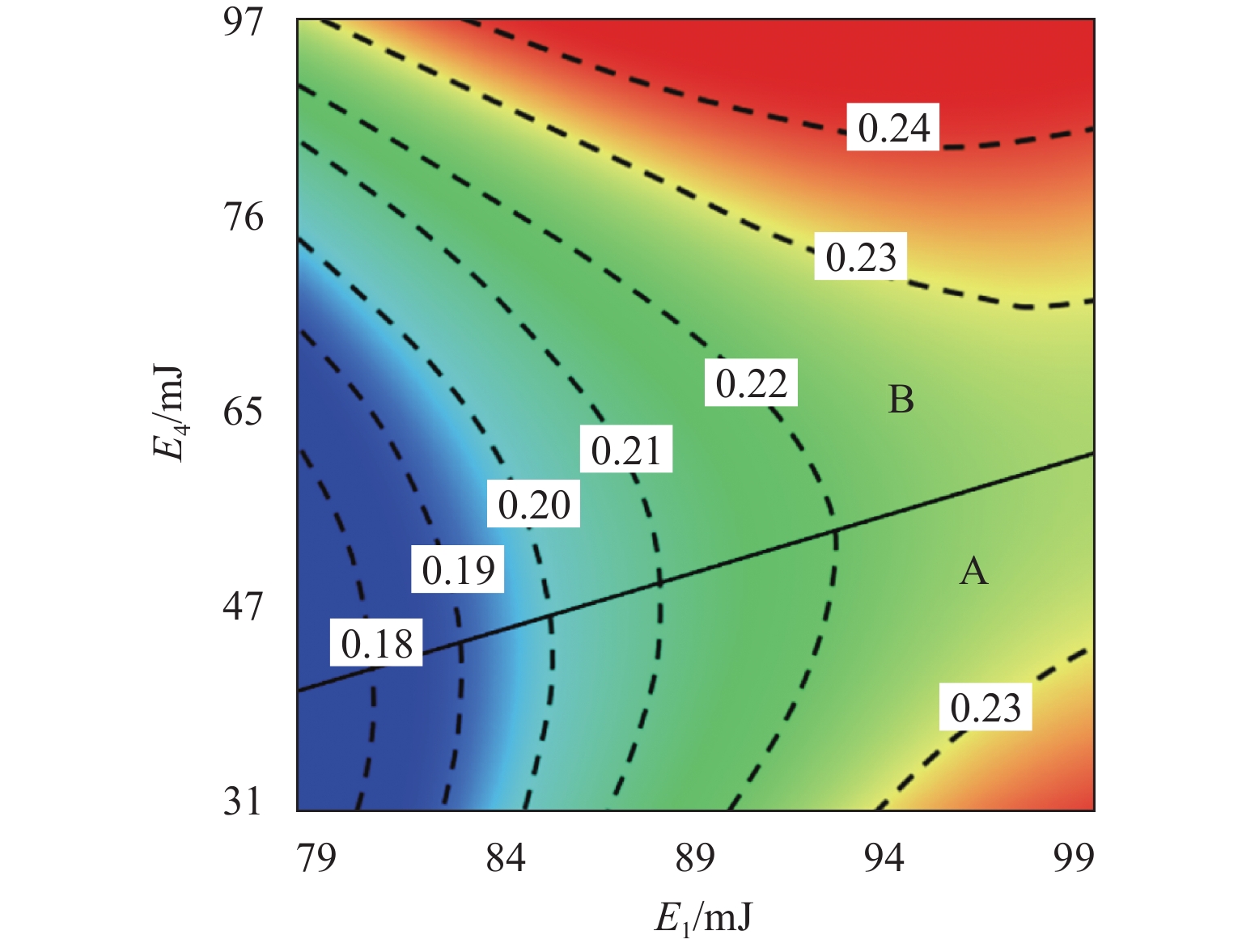
E 1是影响高速电磁阀开启响应时间T o的较敏感参数,T o随着E 1的增加相应地增加了约25%,涡流能量分别与阻尼能量(E 3)和焦耳能量(E 4)之间的交互是影响T o的高敏感参数,同时涡流能量与焦耳能量之间的交互也是影响关闭响应时间T c的高敏感参数;交互参数中起主导作用的因素会随取值范围而改变.Abstract:In order to study the energy distribution inside the high-speed solenoid valve and the coupling relationship between the energy parameters and the dynamic response, the performance of the high-speed solenoid valve was optimized. Firstly, the dynamic model of the high-speed solenoid valve was established based on the finite element method, and the accuracy of the model was validated through the experimental data. Secondly, based on the D-optimal design of experiments and the method of least squares, the response surface prediction model of the solenoid valve’s dynamic response characteristics was constructed, with the energy parameters as the factors. Finally, based on the meta-analysis method, the simulation analysis of the dynamic response of the high-speed solenoid valve with significant energy parameters and parameter interactions was carried out. The results show that the eddy energy
E 1 is the relatively sensitive parameter affecting the response time of high-speed solenoid valve openingT o among the single parameters, andT o increases by about 25% with the increase inE 1. The interaction betweenE 1 and damping energyE 3 and betweenE 1 and Joule energyE 4 is the highly sensitive parameter affectingT o, and the interaction betweenE 1 andE 4 is the highly sensitive parameter affecting the response time of closingT c. The results also find that the factors that play a dominant role in the interaction parameters change with the range of values.-
Key words:
- solenoid valve /
- energy distribution /
- dynamic response /
- response surface method /
- significant factors
-
路径选择建模是交通工程师决策长期交通规划和短期交通管理的重要步骤,在给定道路网络结构和交通需求下,路径选择模型通过解析出行者路径选择机理来推导路段交通流量,同时对交通网络性能进行测试评估.
出行者的路径选择过程不仅受当前网络交通状况(外部因素)的影响,还受出行者自身感知异质性(内部因素)的影响[1]. 根据交通状况影响因素,许多学者采用出行时间和可靠性决策出行路径. Liu等[2]表明出行时间可靠性是交通系统性能中至关重要的影响指标. 在获知网络交通状况后,出行者根据自身感知来决策出行路径[3]. 出行者不会都选择时间最短的出行路径,通常可以接受比最短时间稍长一些的路径. 这是因为:1) 出行者缺乏对出行信息的完全认知;2) 路网系统的不确定性影响出行者做出最优决策;3) 根据以往出行经验,出行者存在惯性心理. Ramos等[4]通过一系列路径选择实验发现,由于出行惯性,在可接受时间延误范围内通勤者不会选择改变自己当前的路径到最短路径上. Zhu等[5]通过GPS和地理信息系统(GIS)数据发现,大部分出行者在选择路径时是有限理性的,他们不一定选择最短路径. Fayyaz等[6]也认为在路径选择过程中,比起出行时间,出行者更关心出行可靠性.
近年来,学者们对出行时间可靠性下的路径选择行为开展了研究. 部分研究把可靠性描述为出行计划中的迟到和早到惩罚;Watling[7]量化通勤出行中迟到惩罚分布,Zhu等[8]在出行效用中融入随机延误,并在弹性通勤中分析出行可靠性. 部分研究运用出行效用的均值和方差来描述出行可靠性;Zhang等[9]采用出行时间预算分析交通需求不确定性,Xu等[10]考虑出行时间偏见,建立弹性需求下的均值超量出行可靠性模型. 与此同时,可以采用鲁棒优化、惯性特征、风险分析、数据统计、网络扰动恢复力测试等方法构建出行时间可靠性下的路径选择模型[11].
由于有限理性可以更准确地描述出行者在路径选择过程中的心理,近几年逐渐成为交通均衡理论的研究热点. Di等[12-15]将有限理性引入到路径选择建模中,揭示了出行者并不会全都选择最短路出行,多于最短路径出行时间一定范围内的道路同样会被选择. Sun等[16]分析了出行者在有限理性出行中使用路径和非使用路径特征. Wang等[17]进一步将有限理性扩展到动态交通均衡.
尽管上述文献运用不同方法对路径选择模型进行了研究,但仍然存在一些不足:1) 传统的路径选择模型缺乏综合考虑出行者有限理性和出行时间可靠性;2) 存在的有限理性均衡模型均认为有限理性阈值是一个已知常数;3) 在建立出行时间和可靠性加权模型时,缺乏对参数估计方法的研究. 为此,本文同时考虑出行时间、出行可靠性和出行者的有限理性,提出双目标用户均衡方法,为了优化解的性质,进一步建立出行可靠性和有限理性下的贝叶斯随机用户均衡模型,设计迭代算法(iterative algorithm,IA)和相继平均算法(method of successive average,MSA)求解,并进行数值仿真实验.
1. 双目标用户均衡模型
在实际出行过程中,出行者往往会根据多个因素来决策出行路径,比如出行时间、出行可靠性等. 采用出行时间预算理论构建出行时间可靠性,数学模型如式(1)所示.
min{Rω,k(T)|P(Tω,k⩽(Eω,k(T)+Rω,k(T)))⩾α}, (1) 式中:
Eω,k(T) 、Rω,k(T) 分别为OD (origin-destination)对ω 第k 条路径的平均出行时间、可靠出行时间,分别如式(2)、(3)所示;ω∈W ,W 为道路网络的OD对集;k∈Kω ,Kω 为OD对ω 所有路径的集合;T=(Tω,k) ,Tω,k 为OD对ω 第k 条路径的出行时间;α 为置信水平;P(•) 为概率函数.Eω,k(T)=∑aδa,ω,k[ta,0+bta,0vna(1−λ1−na)κna(1−λa)(1−n)], (2) Rω,k(T)=Φ−1(α)√∑aδa,ω,kb2t2a,0v2na[1−λ1−2naκ2na(1−λa)(1−2n)−(1−λ1−naκna(1−λa)(1−n))2], (3) 式中:
Φ −1(α) 为标准正态分布的累积分布反函数;va 为路段a上的交通流量,v=(va) ,a∈A ,A 为路段集;ta,0 为路段a自由流速度;δa,ω,k 为路径、路段关联变量,当路径k 包含路段a时,δa,ω,k=1 ,否则,δa,ω,k=0 ;b、n为路段性能函数ta,0[1+b(va/vaκaκa)n] 中的常数,通常b = 0.15 ,n = 4 ;κa 为路段a的容量上界;λa 为路段a的容量上界与下界的比例系数.同时,出行者本身并非完全理性,不一定选择耗时最短的路径,通常出行者可以接受出行时间比最短路稍微长一些的路径. 通常来说,有限理性阈值随着最短出行时间的增加而增加,路程越遥远,出行者可接受的出行时间偏差越大. 同时,该阈值存在一个上界,因为没有出行者可以忍受一个非常大的时间偏差. OD对
ω 的有限理性阈值εω(•) 如式(4)所示,其曲线如图1所示.εω(T)=εmax(1−1exp(σminkEω,k(T))), (4) 式中:
σ 为用户关于最短出行时间的敏感性系数,其值越大,用户对最短出行时间越敏感;εmax 为有限理性阈值的上界.根据出行者出行特征,提出了双目标用户均衡模型,即最小化平均出行时间及出行阈值之和、最小化选择路径的可靠出行时间,如式(5)所示.
{min{Eω,k(T)+εω(T)},minRω,k(T). (5) 2. 出行可靠性和有限理性下的贝叶斯随机用户均衡模型
2.1 多目标出行阻抗
双目标用户均衡模型通常具有多个解,可采用权重法、约束法、动态规划等方法获取双目标模型的帕累托前沿解集,如通过调整权重法的权重系数便可获得模型的最优解集. 从帕累托前沿解集中抓取出与实际居民出行状况最接近的解(交通网络流状态),是城市交通网络规划的基本需求. 由于贝叶斯估计能够同时利用历史经验和当前信息,因此,本文设计了基于贝叶斯统计的权重法来抓取双目标用户均衡模型的最优交通网络流状态. 定义OD对
ω 第k 条路径的多目标出行阻抗为cω,k=Eω,k(T)+εω(T)+βωRω,k(T), (6) 式中:
βω 为OD对ω 的权重系数,β=(βω) .提出的多目标出行阻抗组合了平均出行时间、可靠出行时间和出行者的有限理性,因此,可以更全面地描述了出行者路径选择行为的过程.
2.2 贝叶斯权重系数估计
考虑测量误差e,假设e服从正态分布. 根据城市交通网络流理论,路段流量和出行需求之间的转换关系如式(7)所示.
va=∑ω∑kpω,kδa,ω,kqω+ea, (7) pω,k=exp(−θcω,k)∑kexp(−θcω,k), (8) 式中:
ea 为路段a上的测量误差,e=(ea) ;qω 为OD对ω 的交通需求量;pω,k 为OD对ω 路径k 上的出行者路径选择概率;θ 为出行用户感知误差偏差系数.运用多元正态分布(multivariate normal distribution)描述交通小区的权重系数,并且运用观测路段流量估计权重时,式(8)的分母保持常数. 因此,观测变量与权重之间的关系可以用式(7)、(8)来表达. 随着观测变量的融入,待估变量的后验概率服从多元正态分布[18].
β 的均值向量μY|Z=z 和变量之间的协方差矩阵ΣY|Z=z 分别如式(9)、(10)所示.μY|Z=z=μY+ΣYZΣ−1ZZ(z−μZ), (9) ΣY|Z=z=ΣYY−ΣYZΣ−1ZZΣZY, (10) 式中:Y为所有未观测变量向量(本文中的权重系数);Z为所有观测变量向量,z为其观测值;
μY 、μZ 分别为变量Y、Z的均值向量;ΣYZ 为未观测变量Y和观测变量Z之间的协方差矩阵;ΣZY 、ΣYY 、ΣZZ 均为对应的协方差矩阵.根据观测道路流量值,设计贝叶斯权重系数估计双层模型.
上层模型:
argmaxβfω,k(Z|β)P(β)∫ω,kfω,k(Z|β)P(β)dβ, (11) 约束为式(7)、(8);
下层模型:
fω,k=qωpω,k=qωP(cω,k⩽cω,l,∀l∈Kω,l≠k|cω),∀l∈Kω, (12) 式(11)、(12)中:
fω,k 为OD点对ω 上第k 条路径上的流量;cω 为OD点对ω 上所有路径的多目标出行阻抗向量,即cω=(cω,k) .双层规划模型的上层运用贝叶斯估计获取权重系数
βω ,下层推导出行者路径选择概率pω,k .2.3 均衡模型及解的性质
考虑一个强连通交通网络,分析出行可靠性和有限理性下的贝叶斯随机用户均衡模型状态.
定义1 在出行可靠性和有限理性下的贝叶斯随机用户均衡状态下,仅改变自己的出行路径,出行者不可能减小自身的感知多目标出行阻抗.
式(12)的均衡条件可以等价于:探索到一个路径流量解
f∗∈Ω ,使得对于∀f∗∈Ω ,均满足式(13)所示的变分不等式;Ω 为模型解集.[c(f∗)+(1/θ)lnf∗]T(f−f∗)⩾0,∀f∈Ω, (13) Ω={f|Λf=q,f⩾0,q⩾0}, (14) 式中:
f=(fω,k) ;c(•) 为所有OD点对上所有路径的多目标出行阻抗向量;f∗ 为路径流量均衡解;Λ 为路径、路段的关联矩阵,Λ=(δa,ω,k) ;q=(qω) .式(13)、(14)解的存在性和等价性如定理1、定理2所示.
定理1 (存在性) 出行可靠性和有限理性下的贝叶斯随机用户均衡模型,即式(13)、(14)至少有一个解.
证明 可行集即式(14)是非空、有界闭凸集,且
Eω,k(T) 、εω(T) 和Rω,k(T) 均是关于f 的连续函数,因此,式(13)、(14)至少存在一个解. 证毕.定理2 (等价性) 式(13)、(14)的解与所建模型的均衡解是等价的.
证明 式(13)、(14)等价于
[c(f∗)+(1/1θθ)lnf∗]Tf⩾[c(f∗)+(1/1θθ)lnf∗]Tf∗,∀f∈Ω ,因此,f∗ 是变分不等式的均衡解,当且仅当f∗ 是式(15)的最优解.minf∈Ω[c(f∗)+(1/θ)lnf∗]Tf. (15) 考虑式(15)的KKT (Karush-Kuhn-Tucker)条件,可以得到:
f∗ω,k[cω,k(f∗)+(1/θ)lnf∗ω,k−˜πω]=0, (16) cω,k(f∗)+(1/θ)lnf∗ω,k−˜πω⩾0, (17) f∗ω,k⩾0, (18) 式中:
f∗ω,k 为OD对ω 第k 条路径的流量均衡解;˜πω 为OD对ω 的乘子.由式(16)~(18)发现,变分不等式(式(13)、式(14))的解与均衡条件(式(12))是等价的.
证毕.
3. 模型算法
在求解贝叶斯参数估计时,运用IA算法反复计算上、下层模型,其中,上层的贝叶斯最大后验概率估计运用式(9)、(10)求解,下层模型通过MSA算法计算. 由于所建立的出行可靠性和有限理性下的贝叶斯随机用户均衡模型中多目标出行阻抗非可加,因此,使用路径算法求解.
算法1 IA算法求解贝叶斯参数估计.
步骤1 初始化:收敛精度
ζ 、测量误差e、权重系数β 先验均值向量(同时设初始权重系数值为β(1) )和变量之间的协方差矩阵、观测路段流量Z. 设置初始迭代步数d1=1,下文中,参数上标(d1)表示参数值的第d1次迭代.步骤2 求解下层SUE模型:通过MSA算法求解下层模型,获得关联系数、出行时间均值、方差和有限理性阈值.
步骤3 求解上层贝叶斯参数估计模型:根据式(9)、(10),使用观测路段流量计算权重系数过渡值
ˉβ(d1) .1) 设置观测路段编号s,获取观测路段集维度smax.
2) 根据观测的路段流量zs,运用式(9)、(10)更新权重系数的后验均值
μY|Z=zs 和变量之间的协方差矩阵ΣY|Z=zs ,令观测变量均值μZ|Z=zs=zs ,观测变量间的协方差矩阵ΣZ|Z=zs=0 ,μ(s)=μY|Z=zs ,Σ(s)=ΣY|Z=zs .3) 收敛检验:令
s=s+1 ,如果s⩾smax ,停止计算,令权重系数过渡值ˉβ(d1) 等于更新后的权重均值μ(s) ;否则转到2).步骤4 更新权重系数:令
β(d1+1)=β(d1)+(ˉβ(d1)−β(d1))/1d1d1 .步骤5 收敛检验:如果
‖ ,停止计算,{{\boldsymbol{\beta}} ^{\left( {{d_1}} \right)}} 就是最优的权重系数;否则,令{d_1} = {d_1} + 1 ,转到步骤2.根据最优权重系数值
{{\boldsymbol{\beta}} ^{\left( {{d_1}} \right)}} ,运用算法2求解出行可靠性和有限理性下的贝叶斯随机用户均衡模型.算法2 通过MSA算法求解出行可靠性和有限理性下的贝叶斯随机用户均衡模型.
步骤1 初始化:收敛精度
\nu ,权重系数{\boldsymbol{\beta}} ,可靠出行时间参数\alpha 、{\kappa _a} 、\theta ,有限理性参数{\omega _{\max }} 、\sigma ;设置初始迭代步数{d_2} = 1 . 根据路段流量计算多目标出行阻抗,即式(2)~(4)、(6),当{d_2} = 1 时,路段流量设为0. 根据式(8)计算出行者路径选择概率,并运用式(12)进行流量加载,获得初始路径流量{{\boldsymbol{f}}^{\left( 1 \right)}} .步骤2 更新多目标出行阻抗:根据式(2)~(4)、式 (6)更新多目标出行阻抗
c_{\omega ,k}^{\left( {{d_2}} \right)} .步骤3 搜索方向:根据多目标出行阻抗
c_{\omega ,k}^{\left( {{d_2}} \right)} ,运用式(8)、(12)加载道路流量,计算出辅助路径流量{{\boldsymbol{\bar f}}^{\left( {{d_2}} \right)}} .步骤4 更新路径流量:根据当前路径流量
{{\boldsymbol{f}}^{\left( {{d_2}} \right)}} 和辅助路径流量{{\boldsymbol{\bar f}}^{\left( {{d_2}} \right)}} ,计算搜索方向{{\boldsymbol{\bar f}}^{\left( {{d_2}} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}} ;然后计算新的路径流量{{\boldsymbol{f}}^{\left( {{d_2} + 1} \right)}} = {{\boldsymbol{f}}^{\left( {{d_2}} \right)}} + {{\left( {{{{\boldsymbol{\bar f}}}^{\left( {{d_2}} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right)} \mathord{\left/ {\vphantom {{\left( {{{{\boldsymbol{\bar f}}}^{\left( {{d_2}} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right)} {{d_2}}}} \right. } {{d_2}}} .步骤5 收敛检验:如果
\left\| {{{\boldsymbol{f}}^{\left( {{d_2} + 1} \right)}} - {{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right\|\Big/\left\| {{{\boldsymbol{f}}^{\left( {{d_2}} \right)}}} \right\| \leqslant \nu ,停止计算,f_{\omega ,k}^{\left( {{d_2}} \right)} 就是最优的路径流量;否则,令{d_2} = {d_2} + 1 ,转到步骤2.4. 算例分析
4.1 Nguyen-Dupuis网络
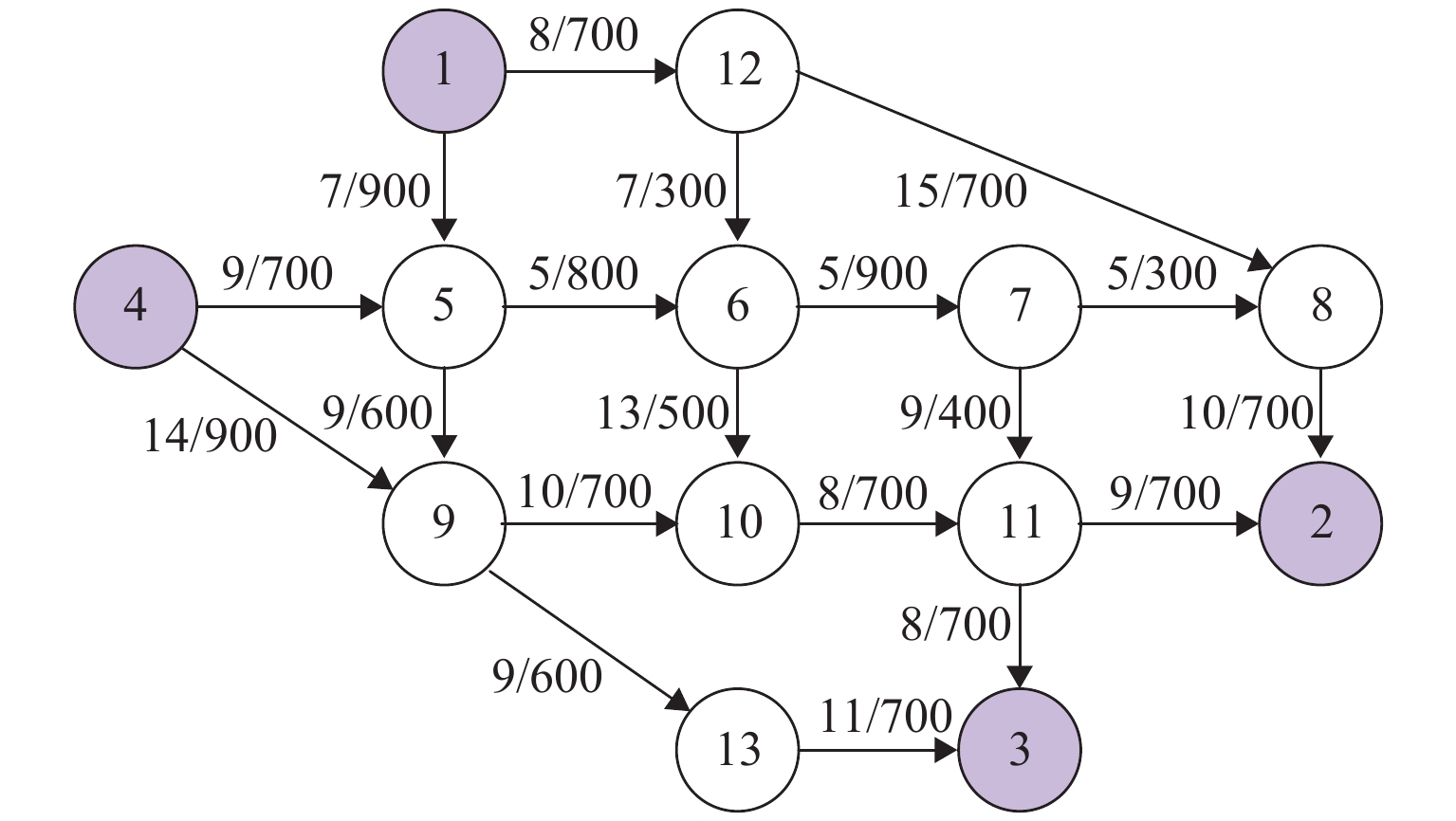
Nguyen-Dupuis网络属性如图2所示,OD对1—2、1—3、4—2、4—3的OD量分别为400、800、600、200辆. 图中:圈中数字为节点编号;有向线段上的数值分别为自由流旅行时间(单位:min)和道路容量(单位:辆/h),例如:节点1—12上的数字“8/700”表示路段自由流旅行时间为8 min,道路容量为700辆/h. 初始化模型中的各参数值:路段容量上界与下界的比例系数
{\lambda _a} = 0.8 ;有限理性阈值上界{\varepsilon _{\max }}{\text{ = 1}} ;敏感系数\sigma = 0.02 ;置信水平\alpha = 0.08 ;出行用户感知误差偏差系数\theta = 1 ;权重系数先验均值向量为(0.25,0.25,0.25,0.25);变量之间的协方差矩阵为diag(0.5,0.5,0.5,0.5).通过计算并分析权重系数的灵敏度,图3测试了贝叶斯权重系数估计的鲁棒性,权重系数的评价指标为
{e_{{\text{RMSE}}}} = \frac{{\left\| {{{\boldsymbol{\beta }}_{{\rm{tru}}}} - {{\boldsymbol{\beta }}_{{\rm{est}}}}} \right\|}}{{\sqrt {\left| \omega \right|} }}, (5) 式中:eRMSE为均方根误差(root mean square error,RMSE);
{{\boldsymbol{\beta }}_{{\text{tru}}}} 、{{\boldsymbol{\beta }}_{{\text{est}}}} 分别为准确的和估计的权重系数向量;\left| \omega \right| 为OD对\omega 的路径数.为了说明建立模型的有效性,使用(2.00,1.50, 2.50,3.00)作为测试网络的“准确权重系数”. 可以发现,在输入需求和观测路段流量扰动都接近0时,估计参数的RMSE达到最小值0.01,表明提出的贝叶斯权重系数估计模型所估计的权重系数值与实际路径选择结构接近. RMSE随着输入需求和观测路段流量扰动的增加而增大,因此,输入参数(路段流量和交通需求)的扰动对权重系数值的估计产生显著影响.
进一步代入估计的权重系数值,对出行可靠性和有限理性下的贝叶斯随机用户均衡模型求解,结果如表1所示. 同上述理论一致,计算得到的解满足均衡条件:仅改变自己的出行路径,出行者不可能减小自身的感知多目标出行阻抗. 例如,在OD对1—2中的第1条路径(即1—12—8—2),根据式(4)、(8)、(12),得到有限理性阈值、选择概率和流量分别为
{\varepsilon _{1 - 2}}= 15[ 1 - 1 / \exp ( 0.02 \times 35.94 ) ] =7.69 min,p_{1 - 2,1}^{} = \exp [ - ( 35.94 + 7.69 + 2 \times 0.52 ) ] \Bigg/{\displaystyle\sum\limits_{k \in {K_{1 \,-\, 2}}} {{\text{exp}}\left( { \;-\; c_{1 \,-\, 2,k}^{}} \right)} } = 0.92,f_{1 - 2,1}^{} = p_{1 - 2,1}^{} {q_{1 - 2}} = 0.920\;6 \times 400 = 368.24 辆,与模型的均衡条件一致.表 1 算例1的模型均衡解Table 1. Equilibrium results of proposed model for case 1OD 路径 时间均值/min 有限理
性阈值/min权重
系数可靠出
行时间/min感知多目标出行阻抗均值/min 流量/辆 1—2 1—12—8—2 35.94 7.69 2.00 0.52 44.67 368.24 1—5—6—7—8—2 38.36 0.70 47.45 23.02 1—5—6—7—11—2 40.35 0.72 49.48 3.02 1—5—6—10—11—2 43.77 0.25 51.96 0.25 1—5—9—10—11—2 44.41 0.20 52.50 0.15 1—12—6—7—8—2 40.04 0.66 49.05 4.65 1—12—6—7—11—2 42.04 0.67 51.07 0.61 1—12—6—10—11—2 45.46 0.07 53.29 0.07 1—3 1—5—6—7—11—3 39.58 8.20 1.50 0.72 48.86 327.15 1—5—6—10—11—3 43.01 0.26 51.60 21.28 1—5—9—10—11—3 43.65 0.21 52.17 12.07 1—12—6—7—11—3 41.27 0.68 50.49 64.53 1—12—6—10—11—3 44.69 0.10 53.04 5.04 1—5—9—13—3 39.85 0.46 48.74 369.93 4—2 4—5—6—7—8—2 39.66 8.21 2.50 0.67 49.55 302.33 4—5—6—7—11—2 41.66 0.69 51.60 39.21 4—5—6—10—11—2 45.08 0.18 53.74 4.66 4—9—10—11—2 41.41 0.05 49.75 250.74 4—5—9—10—11—2 45.72 0.09 54.16 3.05 4—3 4—5—6—7—11—3 40.89 7.82 3.00 0.70 50.81 1.40 4—5—6—10—11—3 44.31 0.19 52.70 0.21 4—5—9—10—11—3 44.95 0.11 53.10 0.14 4—9—13—3 36.84 0.42 45.92 184.53 4—5—9—13—3 41.15 0.42 50.23 2.43 4—9—10—11—3 40.64 0.08 48.70 11.30 4.2 Sioux Falls网络
进一步将模型运用在Sioux Falls网络中,该路网由24个结点、76个路段和550个OD对组成,路段特性和OD需求见文献[19],其余参数设置与Nguyen-Dupuis网络算例一致. 图4绘制了IA算法和贝叶斯更新策略的收敛过程,其中,贝叶斯更新策略为IA算法的第5次迭代过程. 可以发现:运行15 s后,RMSE到0.05;迭代过程中权重系数的RMSE值稳定下降,表明算法具有良好的鲁棒性.
图5测试了求解出行可靠性和有限理性下的贝叶斯随机用户均衡模型算法的收敛速度,可以发现:设计的MSA算法能够快速收敛,在1 s内精度可达到10−6.
选取OD对11—6的5条路径(路径1~5)作为测试对象,图6展示了模型平均出行时间、有限理性阈值、可靠出行时间和路径流量的迭代过程和快速收敛性;同时可以验证算法收敛至模型均衡解,例如,在OD对11—6的第1条路径中,根据式(4)、式(8)、(12),计算出有限理性阈值、选择概率和路径流量分别为
{\varepsilon _{11 - 6}} = 15\left[ {1 - {1 \mathord{\left/ {\vphantom {1 {\exp \left( {0.02 \times 15.17} \right)}}} \right. } {\exp \left( {0.02 \times 15.17} \right)}}} \right] = 3.93 min,p_{11 - 6,1}^{} = \exp \left[ { - \left( {16.20 + 3.93 + 1.3 \times 0.15} \right)} \right] \left/ \right. {{} \mathord{ {\vphantom {{} {\sum\limits_{k \in {K_{11 - 6}}} {{\text{exp}}\left( { - c_{11 - 6,k}^{}} \right)} }}} } {\displaystyle\sum\limits_{k \in {K_{11 - 6}}} {{\text{exp}}\left( { - c_{11 - 6,k}^{}} \right)} }} = 0.26 ,f_{11 - 6,1}^{} = p_{11 - 6,1}^{}{q_{11 - 6}} = 0.225\;9\times 320 =72.29 辆. 因此,模型解收敛至均衡条件.5. 结 论
1) 出行者会同时根据出行时间、出行可靠性和自身的有限理性来选择出行路径,从而提出了双目标用户均衡模型. 在目标一中,用户追求有限理性范围内出行时间最短;在目标二中,用户同时期望最短的可靠出行时间. 为克服双目标模型多解的问题,进一步运用变分不等式建立了出行可靠性和有限理性下的贝叶斯随机用户均衡模型,该模型通过添加权重系数来统一化两个出行目标,并采用基于双层规划框架的贝叶斯方法估计权重系数. 分别设计了IA算法和MSA算法计算贝叶斯参数估计和变分不等式模型.
2) 数值实验表明,提出的贝叶斯权重系数估计模型能够推断精度较高且具有鲁棒性的权重系数值;输入参数的扰动对权重系数估计值产生显著影响,当扰动都接近0的时候,权重系数的均方根误差达到最小值0.01;设计的IA算法和MSA算法具有良好的鲁棒性,且可以快速收敛于均衡解,IA算法在运行15 s后均方根误差达到0.05,MSA算法在1 s时精度达到10−6.
3) 后续将在实际城市路网中进行模型应用,为交通规划提供数据参考;同时进一步将模型应用到拥堵收费、车辆限速等网络设计问题上.
-
表 1 高速电磁阀不同阶段能量分布
Table 1. Energy distribution of high-speed solenoid valves at different stages
% 能量 打开阶段 保持阶段 关闭阶段 E1 70.87 57.41 37.05 E2 3.93 17.06 19.03 E3 2.37 25.53 9.69 E4 14.56 0 0 E5 8.27 0 34.23 表 2 参数变化范围
Table 2. Parameter variation range
改变参数 基准值 变化范围 电导率/(S·mm−1) 2000 1400 ~2600 弹簧刚度/(N·mm−1) 57 47~67 阻尼系数/(N·(m·s−1)−1) 95 75~115 电阻/Ω 0.38 0.18~0.58 表 3 仿真计算矩阵
Table 3. Simulation calculation matrix
组别 E1/J E2/J E3/J E4/J To/ms Tc/ms 1 0.099 0.014 0.00044 0.033 0.32 0.22 2 0.086 0.016 0.00019 0.031 0.28 0.19 3 0.098 0.018 0.00022 0.032 0.34 0.23 4 0.090 0.018 0.00037 0.033 0.30 0.21 5 0.086 0.012 0.00095 0.031 0.29 0.22 6 0.097 0.012 0.00035 0.032 0.37 0.27 7 0.097 0.016 0.00030 0.032 0.37 0.26 8 0.086 0.018 0.00028 0.031 0.30 0.23 9 0.099 0.012 0.00028 0.049 0.34 0.22 10 0.085 0.012 0.00072 0.047 0.29 0.21 11 0.095 0.018 0.00041 0.048 0.35 0.24 12 0.092 0.014 0.00092 0.049 0.31 0.23 13 0.087 0.012 0.00053 0.077 0.30 0.21 14 0.082 0.018 0.00059 0.075 0.29 0.20 15 0.091 0.016 0.00022 0.079 0.35 0.23 16 0.095 0.014 0.00017 0.080 0.38 0.25 17 0.082 0.012 0.00079 0.075 0.31 0.23 18 0.079 0.012 0.00053 0.088 0.29 0.22 19 0.092 0.018 0.00004 0.093 0.36 0.22 20 0.092 0.012 0.00014 0.093 0.37 0.24 21 0.079 0.014 0.00057 0.088 0.30 0.21 22 0.085 0.016 0.00054 0.091 0.32 0.23 23 0.093 0.012 0.00045 0.097 0.36 0.26 24 0.079 0.018 0.00041 0.088 0.32 0.23 25 0.092 0.018 0.00006 0.093 0.40 0.26 26 0.092 0.018 0.00006 0.093 0.40 0.26 27 0.092 0.018 0.00006 0.093 0.40 0.26 28 0.092 0.018 0.00006 0.093 0.40 0.26 表 4 能量参数对响应时间的敏感程度
Table 4. Sensitivity of energy parameters to response time
参数 开启响应时间的 μ* 关闭响应时间的 μ* E1 0.096 0.056 E2 0.007 0.014 E3 0.032 0.012 E4 0.052 0.034 E1E2 0.032 0.112 E1E3 0.491 0.135 E1E4 0.249 0.253 E2E3 0.016 0.099 E2E4 0.077 0.018 E3E4 0.019 0.018 -
[1] GU Y Q, FAN L Y, ZHANG J Y, et al. Multi-objective optimization of high-speed solenoid valve for biodiesel electronic unit pump[J]. Current Chinese Science, 2022, 1(1): 38-47. [2] TAO G, CHEN H Y, J Y Y, et al. Optimal design of the magnetic field of a high-speed response solenoid valve[J]. Journal of Materials Processing Technology, 2002, 129(1/2/3): 555-558. [3] WANG Q L, YANG F Y, YANG Q, et al. Experimental analysis of new high-speed powerful digital solenoid valves[J]. Energy Conversion and Management, 2011, 52(5): 2309-2313. doi: 10.1016/j.enconman.2010.12.032 [4] SUN Z Y, LI G X, WANG L, et al. Effects of structure parameters on the static electromagnetic characteristics of solenoid valve for an electronic unit pump[J]. Energy Conversion and Management, 2016, 113: 119-130. doi: 10.1016/j.enconman.2016.01.031 [5] ZHAO J H, WANG M L, WANG Z J, et al. Different boost voltage effects on the dynamic response and energy losses of high-speed solenoid valves[J]. Applied Thermal Engineering, 2017, 123: 1494-1503. doi: 10.1016/j.applthermaleng.2017.05.117 [6] BREIDI F, HELMUS T, LUMKES J. The impact of peak-and-hold and reverse current solenoid driving strategies on the dynamic performance of commercial cartridge valves in a digital pump/motor[J]. International Journal of Fluid Power, 2016, 17(1): 37-47. doi: 10.1080/14399776.2015.1120138 [7] EBRAHIMI N, SCHIMPF P, JAFARI A. Design optimization of a solenoid-based electromagnetic soft actuator with permanent magnet core[J]. Sensors and Actuators A: Physical, 2018, 284: 276-285. doi: 10.1016/j.sna.2018.10.026 [8] ZHAO J H, YUE P F, GREKHOV L, et al. Hold current effects on the power losses of high-speed solenoid valve for common-rail injector[J]. Applied Thermal Engineering, 2018, 128: 1579-1587. doi: 10.1016/j.applthermaleng.2017.09.123 [9] LAN Q, BAI Y, FAN L Y, et al. Investigation on fuel injection quantity of low-speed diesel engine fuel system based on response surface prediction model[J]. Energy, 2020, 211: 118946.1-118946.11. [10] MÄKELÄ M. Experimental design and response surface methodology in energy applications: a tutorial review[J]. Energy Conversion and Management, 2017, 151: 630-640. doi: 10.1016/j.enconman.2017.09.021 [11] VITALI R. Response surface methods for high-dimensional structural design problems[M]. Florida: University of Florida, 2000: 270-289. [12] GE Q, MENENDEZ M. Extending Morris method for qualitative global sensitivity analysis of models with dependent inputs[J]. Reliability Engineering & System Safety, 2017, 162: 28-39. [13] FENG K X, LU Z Z, XIAO S N. A new global sensitivity measure based on the elementary effects method[J]. Computers & Structures, 2020, 229: 106183.1-106183.12. [14] FENG K X, LU Z Z, YANG C Q. Enhanced Morris method for global sensitivity analysis: good proxy of Sobol’ index[J]. Structural and Multidisciplinary Optimization, 2019, 59(2): 373-387. doi: 10.1007/s00158-018-2071-7 -





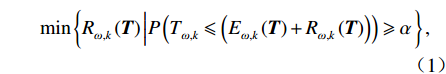
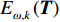

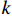
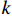
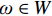
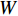
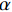
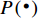
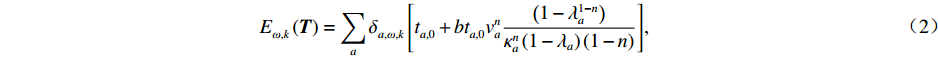

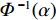
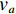
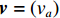
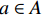
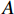
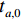
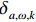
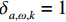
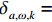
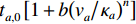
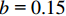

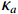
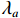
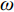
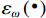

 下载:
下载:
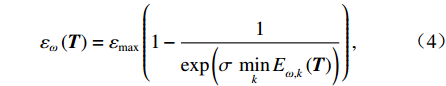
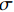

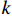
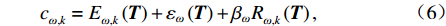
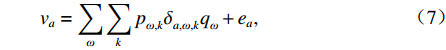
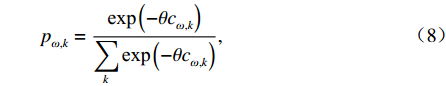
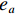

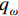
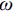
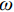
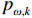
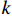
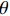
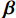
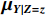
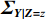
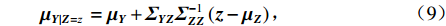
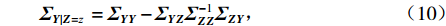
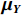

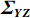
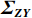


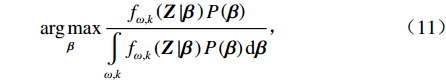
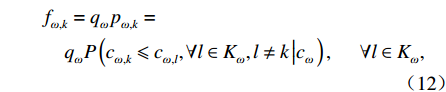
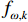
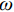
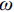
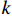
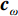
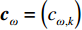
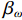
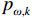
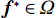
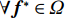

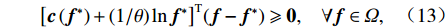
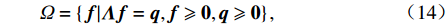
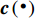
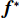
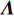
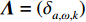
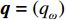
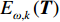
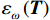
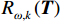
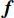
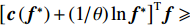
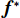
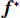
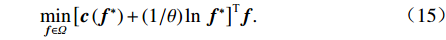
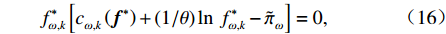
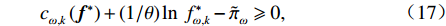

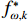
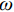
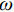

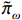
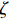
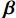
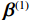
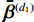
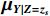
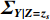
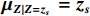

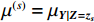
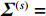
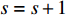

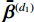
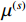
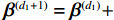
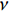
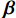
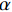
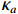
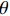

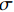
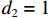
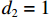
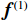
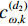
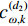
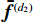
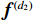
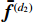
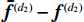
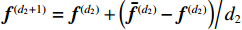
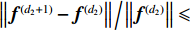
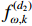
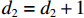
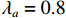
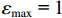
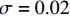
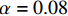
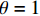


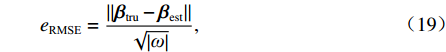
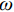
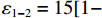
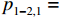
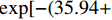
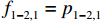



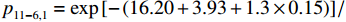
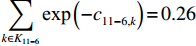
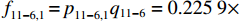


 下载:
下载: