Damage Analysis of Long-Span Continuous Beam Bridges Under Strong Earthquakes
-
摘要:
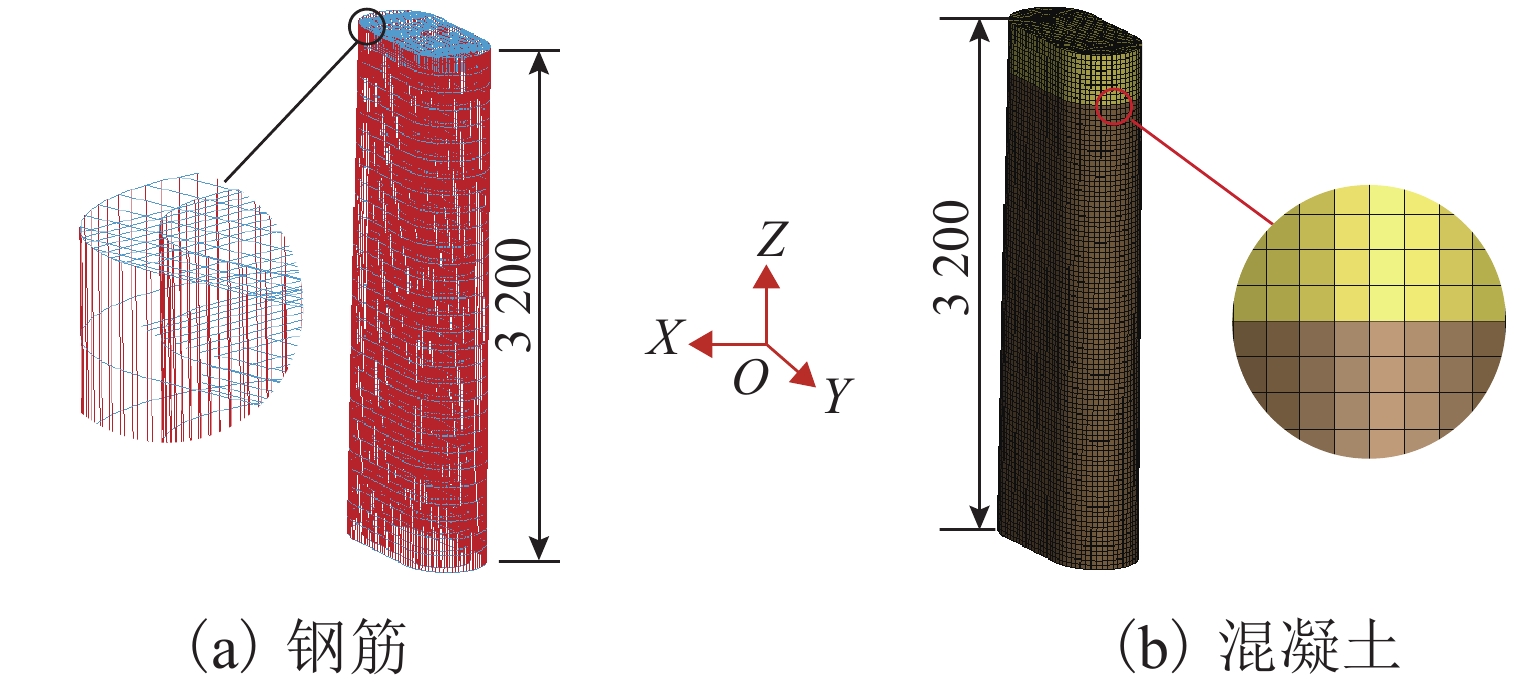
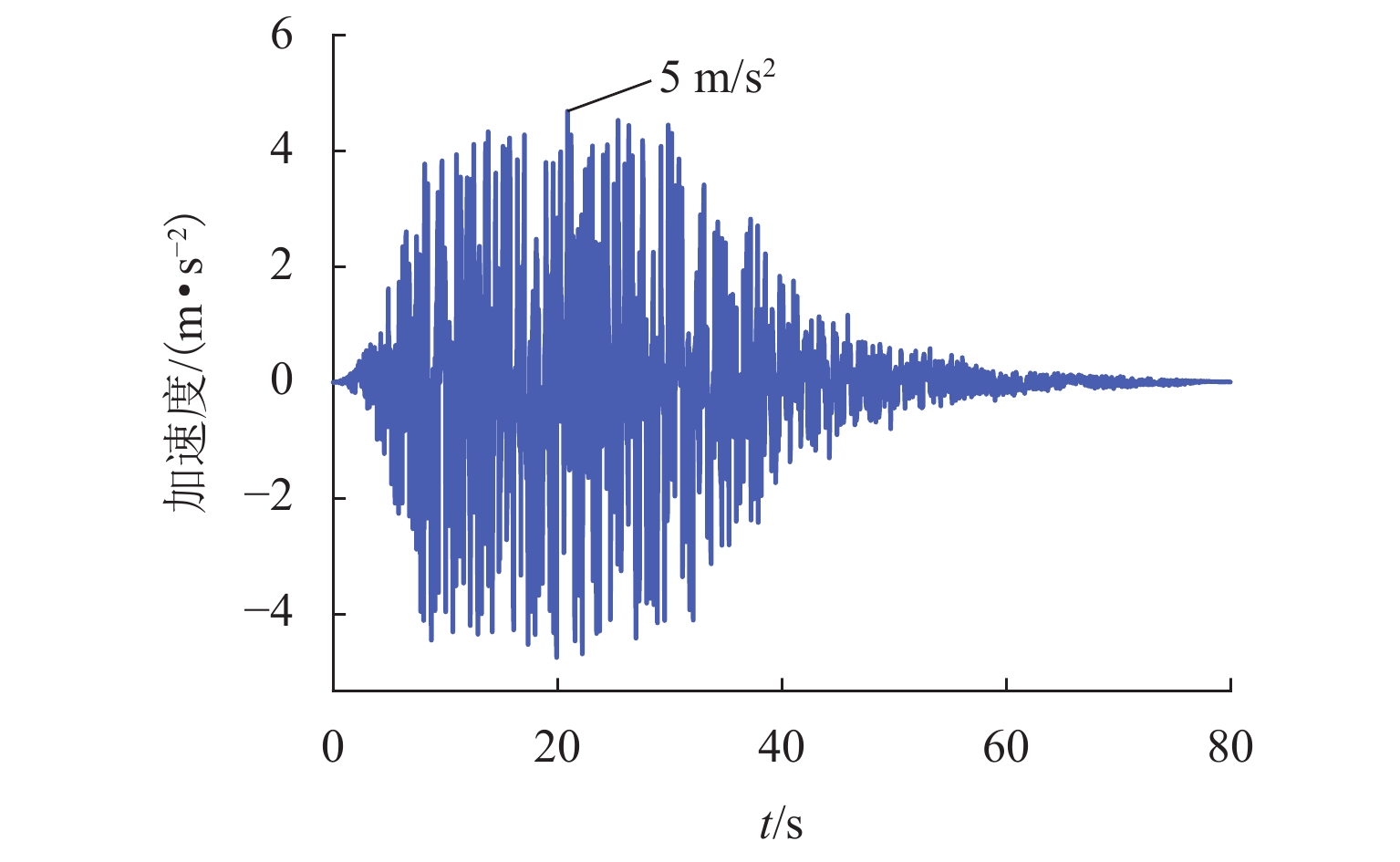
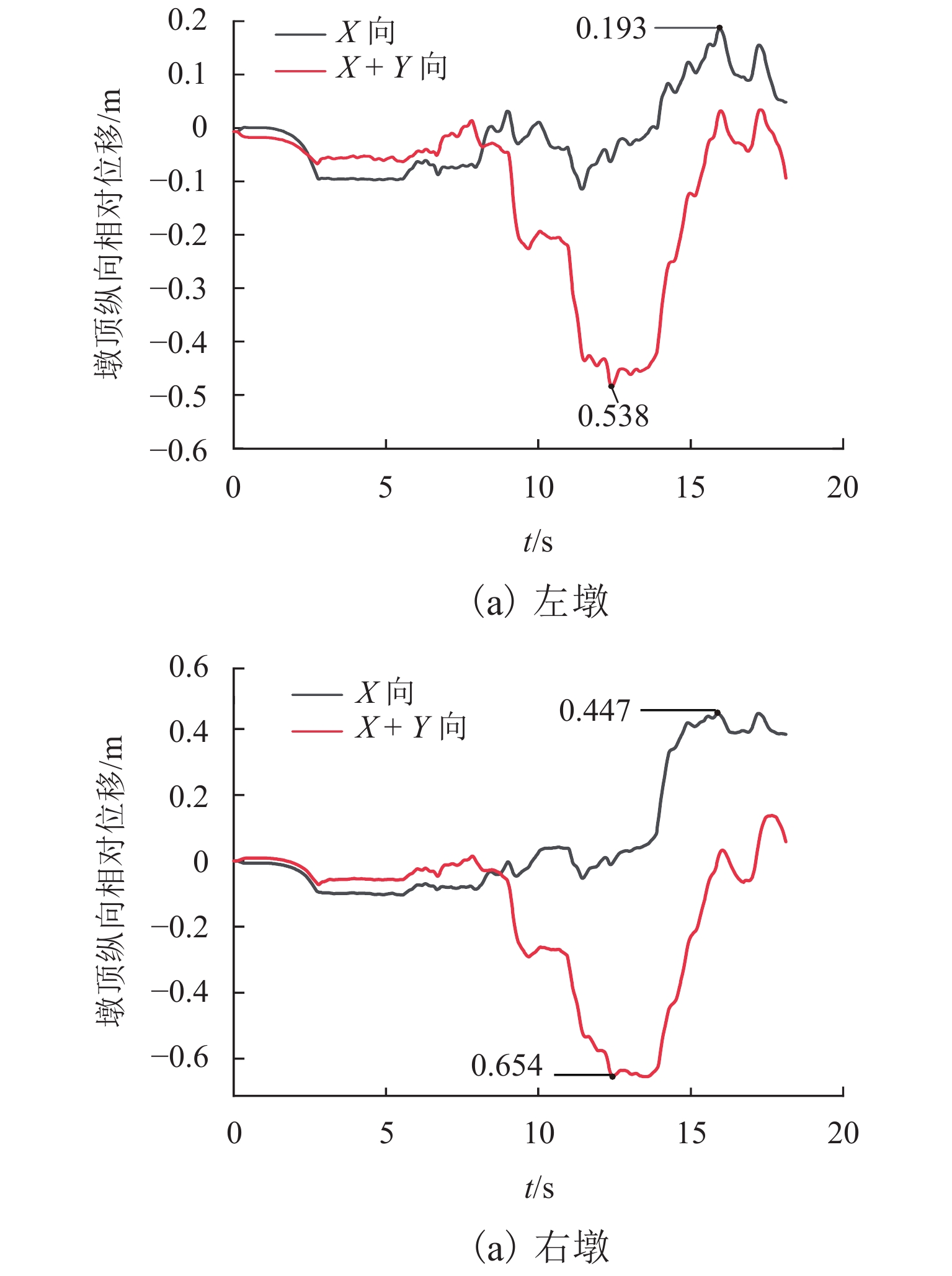
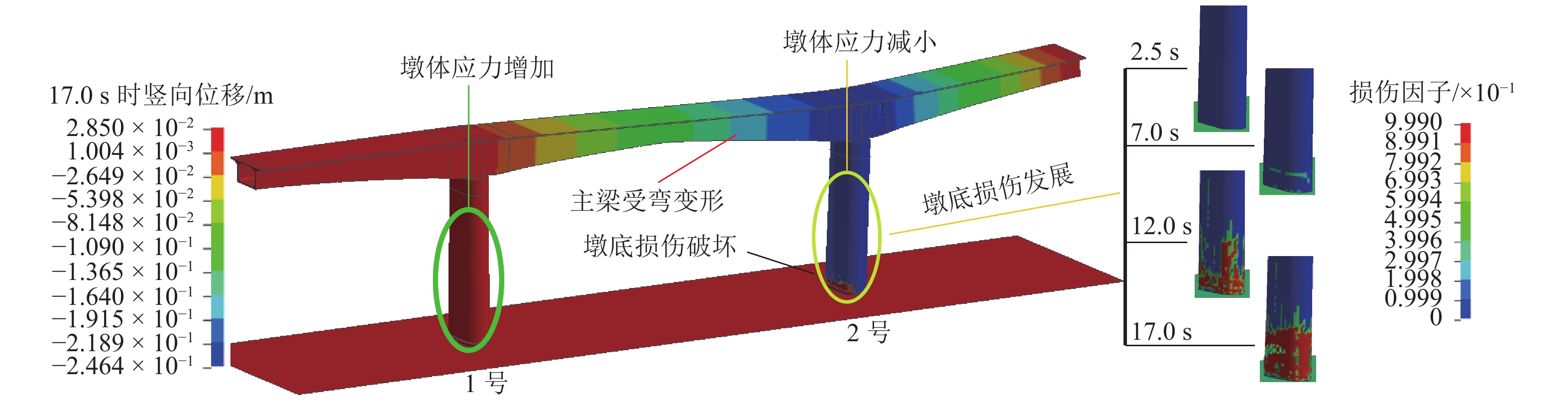
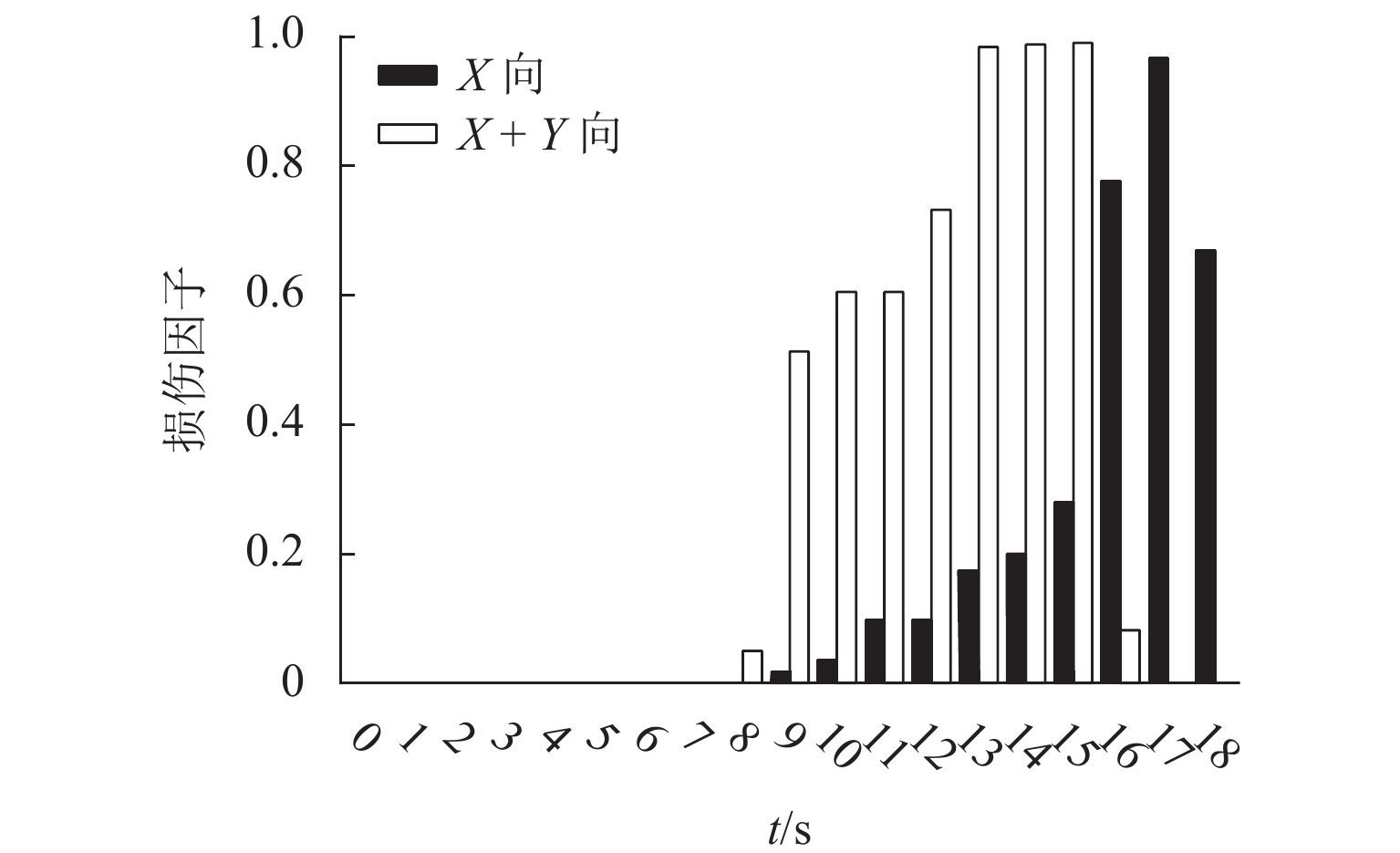
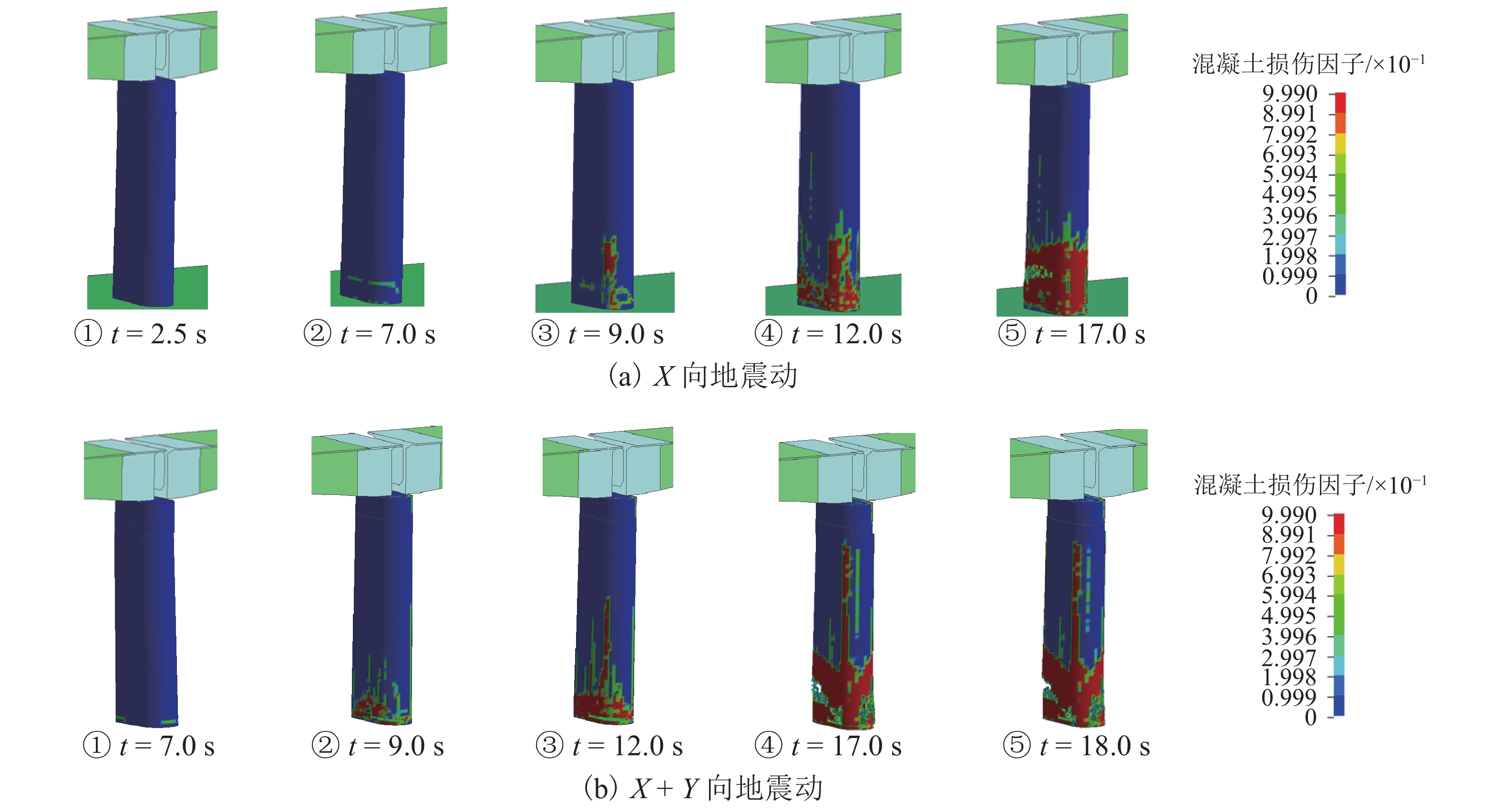
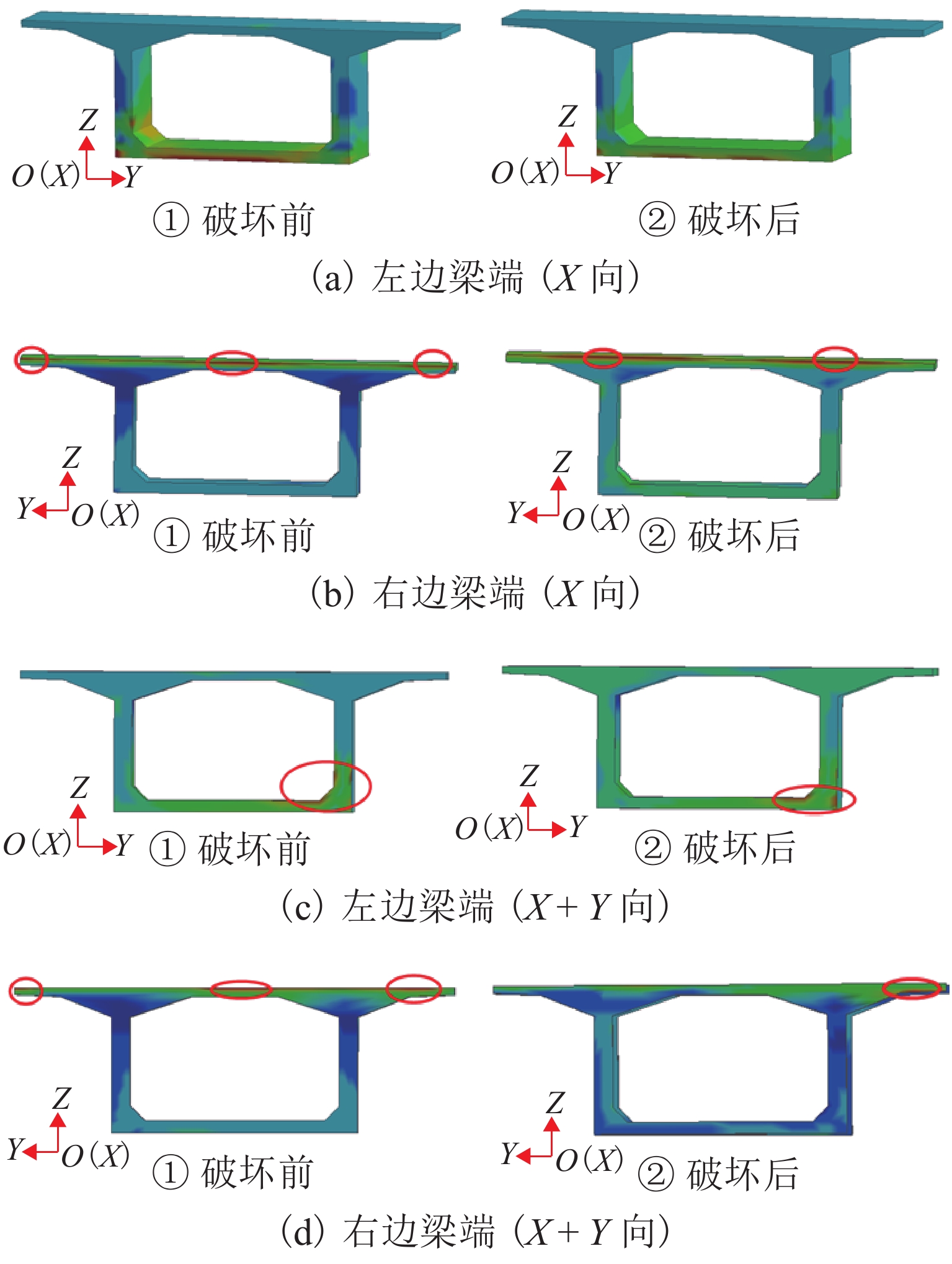
连续梁桥结构常因地震发生损伤甚至倒塌而失去交通作用，研究强震下大跨度连续梁桥的损伤破坏机制对提高桥梁抗倒塌性能具有重要意义. 基于有限元软件ANSYS/LS-DYNA，考虑桥墩材料非线性、损伤过程大变形非线性以及梁端非线性碰撞，建立大跨度连续梁桥在强震作用下的损伤三维数值模型，进行非线性分析，直观模拟大跨度连续梁桥在强震作用下的损伤破坏过程，从连续梁桥的应变与位移响应、桥墩损伤和梁-台间碰撞作用等方面分析了大跨度连续梁桥的地震损伤情况. 研究结果表明：单向地震动输入与双向地震动输入作用下破坏模式基本一致，破坏模式由桥梁结构本身决定，地震动输入方式影响较小；大跨度连续梁桥的地震损伤是逐渐发展的过程，桥墩混凝土损伤因子不断累积达到0.99，固定墩底部发生受弯塑性破坏，桥梁发生损伤破坏.
Abstract:The continuous beam bridge is often damaged or even collapses due to the earthquake and thus loses its traffic function. Therefore, it is important to study the damage mechanism of large-span continuous beam bridges under strong earthquakes to improve the bridge collapse resistance. Based on the finite element software ANSYS/LS-DYNA, a three-dimensional numerical model of the damage of a large-span continuous beam bridge under strong earthquakes was established, which considered the material nonlinearity of the bridge pier, the large deformation nonlinearity of the damage process, and the nonlinear collision of the beam end. In addition, nonlinear analysis was performed to visually simulate the damage process of the large-span continuous beam bridge under strong earthquakes. The seismic damage of the large-span continuous beam bridge was analyzed in terms of strain and displacement response, pier damage, and girder-platform collision. The study results show that the damage modes of the one-way ground motion input and two-way ground motion input are basically the same, and the damage mode is determined by the bridge structure, and the ground motion input mode has less influence; the seismic damage of the large-span continuous beam bridge involves a gradual development process; the concrete damage factor of the bridge pier accumulates to 0.99; the bending plastic damage occurs at the bottom of the fixed pier, and the bridge is damaged.
-
Key words:
- long-span continuous beam bridges /
- earthquake effect /
- failure mode /
- damage analysis /
- pier damage
-
图 5 墩顶与主梁纵向相对位移
Figure 5. Longitudinal relative displacement of pier top and main beam
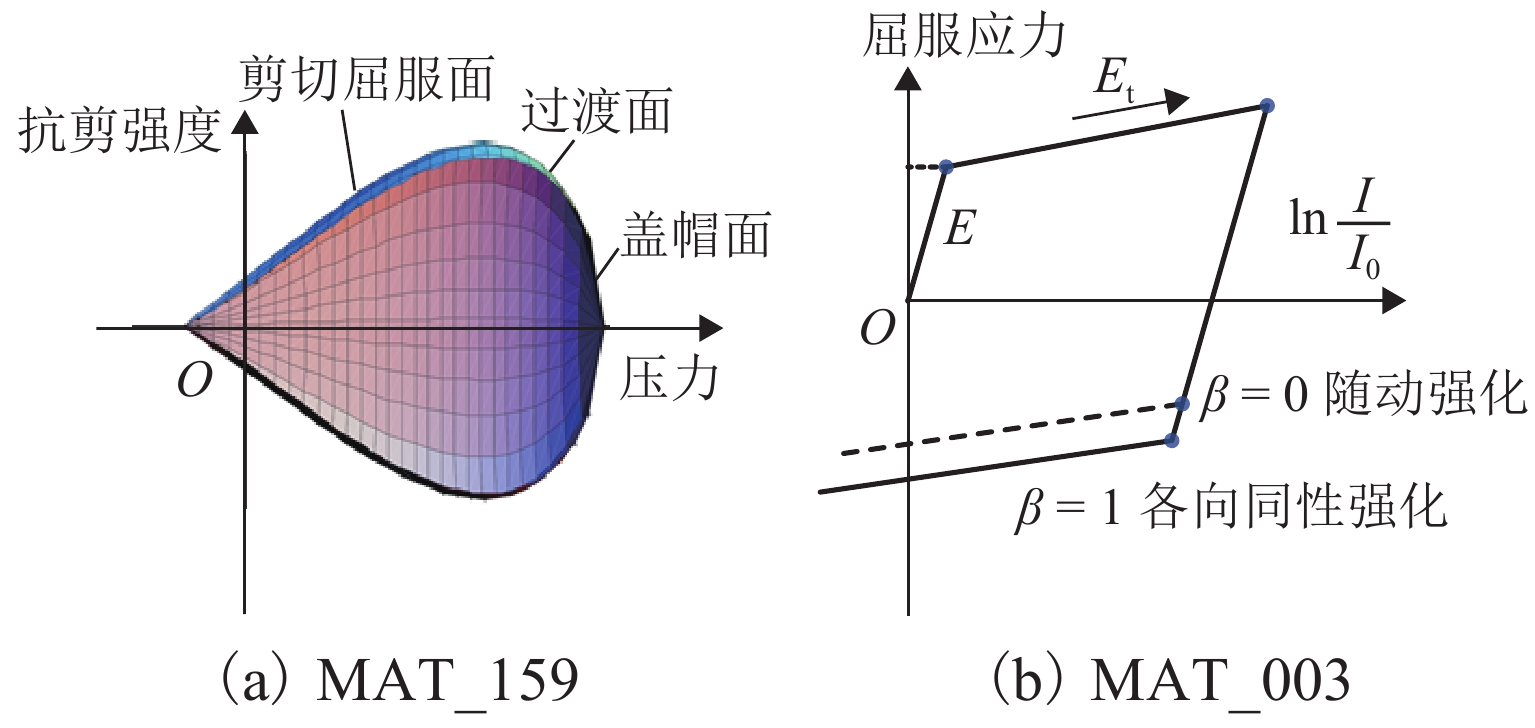
表 1 混凝土模型部分参数
Table 1. Partial parameters of concrete model
参数 等级 密度/
（kg·m−3）抗压强度/
GPa骨料
直径/m侵蚀破坏
参数取值 C50 2400 50 0.02 1.1 
下载: 导出CSV
表 2 钢筋模型部分参数
Table 2. Partial parameters of steel bar model
参数 泊松比 密度/
（ kg·m−3）屈服应力/
GPa弹性模量/
GPa失效应变 取值 0.3 7800 400 200000 0.12
下载: 导出CSV
表 3 桥梁动力特性
Table 3. Bridge dynamic characteristics
阶数 频率/Hz 振型特点 1 1.55 主梁第 1 阶纵向振动 2 2.82 主梁第 1 阶横向振动 3 3.02 主梁第 2 阶横向振动 4 3.53 主梁跨中横向振动 5 3.61 主梁第 1 阶竖向振动 6 4.80 主梁第 2 阶纵向振动 7 5.84 主梁第 1 阶反对称横向振动 8 7.70 主缆第 1 阶对称横向振动 9 7.74 主梁第 1 阶反对称竖向振动 10 8.38 主梁第 1 阶对称竖向振动
下载: 导出CSV
-
[1] 游四方,郑史雄,贾宏宇,等. 地震作用下简支梁桥纵向碰撞模拟[J]. 铁道标准设计,2020,64(12): 59-66.YOU Sifang, ZHENG Shixiong, JIA Hongyu, et al. Longitudinal colliding simulation of simply supported beam bridges under ground motions[J]. Railway Standard Design, 2020, 64(12): 59-66. [2] 刘磊,赵东升,朱瑜,等. 1993—2017年我国大陆地震灾害损失的时空特征[J]. 自然灾害学报,2021,30(3): 14-23.LIU Lei, ZHAO Dongsheng, ZHU Yu, et al. Spatiotemporal characteristics of earthquake hazard losses in mainland China during 1993−2017[J]. Journal of Natural Disasters, 2021, 30(3): 14-23. [3] HAO H, TANG E K C. Numerical simulation of a cable-stayed bridge response to blast loads, partⅡ: damage prediction and FRP strengthening[J]. Engineering Structures., 2010, 32(10): 3193-3205. doi: 10.1016/j.engstruct.2010.06.006 [4] 谢文,孙利民. 采用耗能辅助墩的超大跨斜拉桥顺桥向地震损伤控制[J]. 中南大学学报(自然科学版),2013,44(11): 4672-4681.XIE Wei, SUN Limin. Seismic damage control of long span cable-stayed bridges by supporting piers with energy dissipating in longitudinal direction[J]. Journal of Central South University (Science and Technology), 2013, 44(11): 4672-4681. [5] 仇清良,仇步云. 强震作用下大跨度连续梁桥的倒塌破坏研究[J]. 工程抗震与加固改造,2014,36(1): 57-60,134.QIU Qingliang, QIU Buyun. Study on the collapse and failure of long-span continuous beam bridge under strong earthquake[J]. Earthquake Resistant Engineering and Retrofitting, 2014, 36(1): 57-60,134. [6] 黎雅乐,宗周红,黄学漾,等. 强震下钢筋混凝土连续梁桥非线性动力响应分析[J]. 东南大学学报(自然科学版),2016,46(6): 1271-1277.LI Yale, ZONG Zhouhong, HUANG Xueyang, et al. Nonlinear dynamic response analysis of reinforced concrete continuous girder bridge under strong earthquake excitations[J]. Journal of Southeast University (Natural Science Edition), 2016, 46(6): 1271-1277. [7] 左烨,孙广俊,李鸿晶. 混凝土梁桥地震倒塌失效机制[J]. 南京工业大学学报(自然科学版),2018,40(3): 73-80,104.ZUO Ye, SUN Guangjun, LI Hongjing. Failure mechanism of concrete girder bridges collapse during earthquakes[J]. Journal of Nanjing University of Technology (Natural Science Edition), 2018, 40(3): 73-80,104. [8] 王学伟. 公铁两用斜拉桥强地震作用下的连续倒塌过程数值模拟[J]. 西南公路,2018,147(3): 143-148. [9] 黎雅乐,宗周红,黄学漾,等. 基于倒塌分析的连续梁桥地震损伤评估方法[J]. 振动. 测试与诊断,2019,39(4): 867-874,911.LI Yale, ZONG Zhouhong, HUANG Xueyang, et al. Collapse analysis-based seismic damage evaluation of continuous girder bridge[J]. Journal of Vibration, Measurement & Diagnosis, 2019, 39(4): 867-874,911. [10] 陈敬一,杜修力,韩强,等. 摇摆双层桥梁地震反应及抗倒塌能力分析[J]. 工程力学,2020,37(10): 56-69.CHEN Jingyi, DU Xiuli, HAN Qiang, et al. Analysis of seismic response and overturning resistance of rocking double-deck bridge system[J]. Engineering Mechanics, 2020, 37(10): 56-69. [11] 周艳,张雷明,刘西拉. 美国Cypress高架桥地震倒塌的仿真分析[J]. 岩石力学与工程学报,2005,24(17): 3035-3044.ZHOU Yan, ZHANG Leiming, LIU Xila. Collapse simulation and analysis of Cypress viaduct during Loma Prieta earthquake[J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(17): 3035-3044. [12] 徐俊祥,刘西拉. 地裂时桥梁倒塌过程研究[J]. 中国铁道科学,2008,29(1): 17-21.XU Junxiang, LIU Xila. Study on bridge collapse resulting from fault rupture[J]. China Railway Science, 2008, 29(1): 17-21. [13] JOHNSON N, RANF R T, SAIIDI M S, et al. Seismic testing of a two-span reinforced concrete bridge[J]. Journal of Bridge Engineering, 2008, 13(2): 173-182. doi: 10.1061/(ASCE)1084-0702(2008)13:2(173) [14] MURRAY Y D, LEWIS B A. Numerical simulation of damage in concrete[R]. Colorado Springs: APTEK, Inc., 1995. [15] SIMO J C, JU J W. Strain-and stress-based continuum damage models—I. formulation[J]. International Journal of Solids and Structures, 1987, 23(7): 821-840. doi: 10.1016/0020-7683(87)90083-7 [16] MURRAY Y D. Users manual for LS-DYNA concrete material model 159, FHWA-HRT-05-062[R]. Colorado Springs: APTEK, Inc., 2007. [17] BRUGGI M. Generating strut-and-tie patterns for reinforced concrete structures using topology optimization[J]. Computers & Structures, 2009, 87(23/24): 1483-1495. [18] Livermore Software Technology Corporation. LS-DYNA theory manual[M]. Livermore: Livermore Software Technology Corporation, 2019. [19] 张沧海. 大跨度桥梁多向多点激励地震反应分析[D]. 哈尔滨: 中国地震局工程力学研究所, 2011. [20] MUTHUKUMAR S, DESROCHES R. A Hertz contact model with non-linear damping for pounding simulation[J]. Earthquake Engineering & Structural Dynamics, 2006, 35(7): 811-828. -
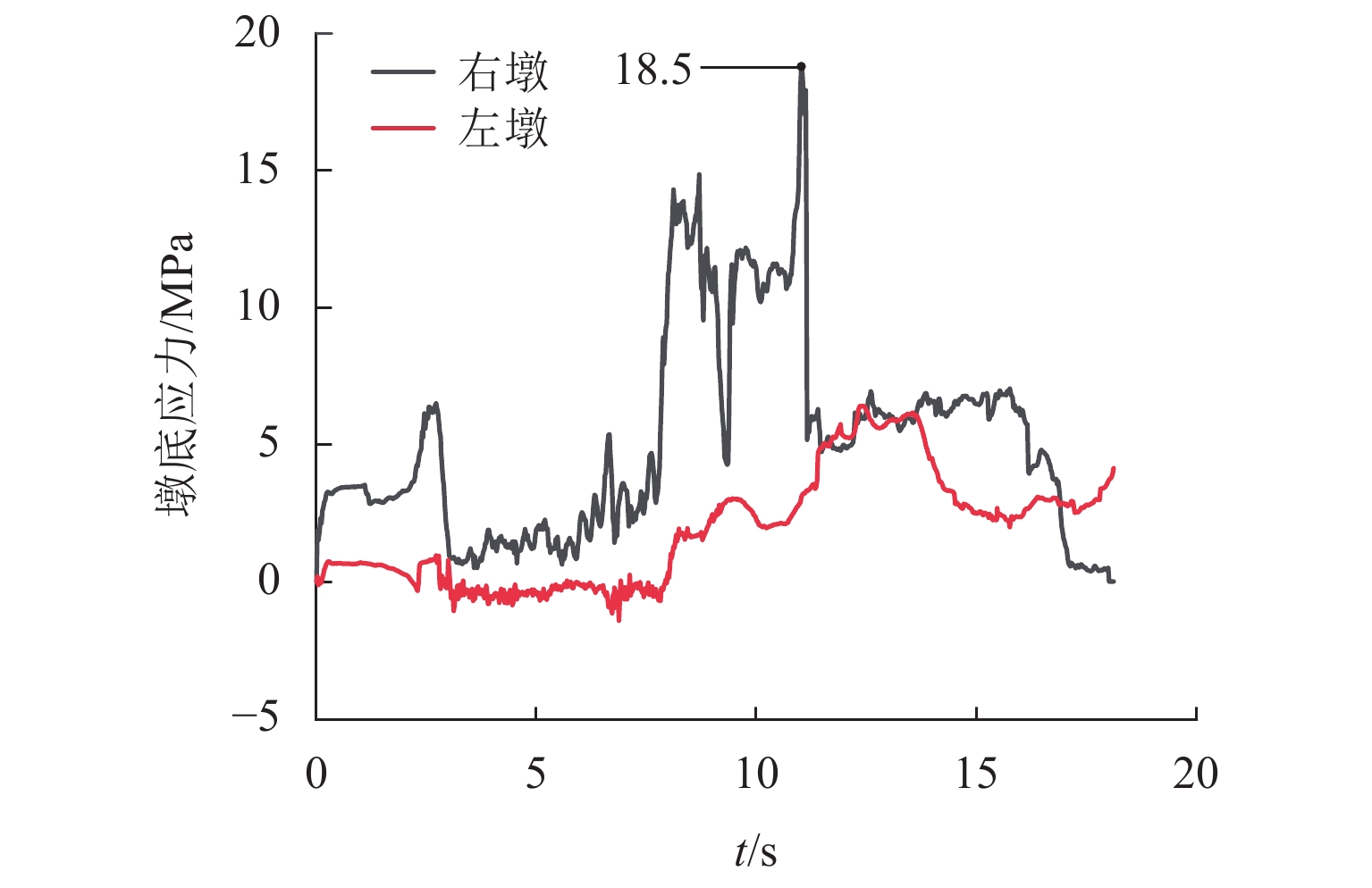
下载:
点击查看大图
计量
- 文章访问数: 316
- HTML全文浏览量: 65
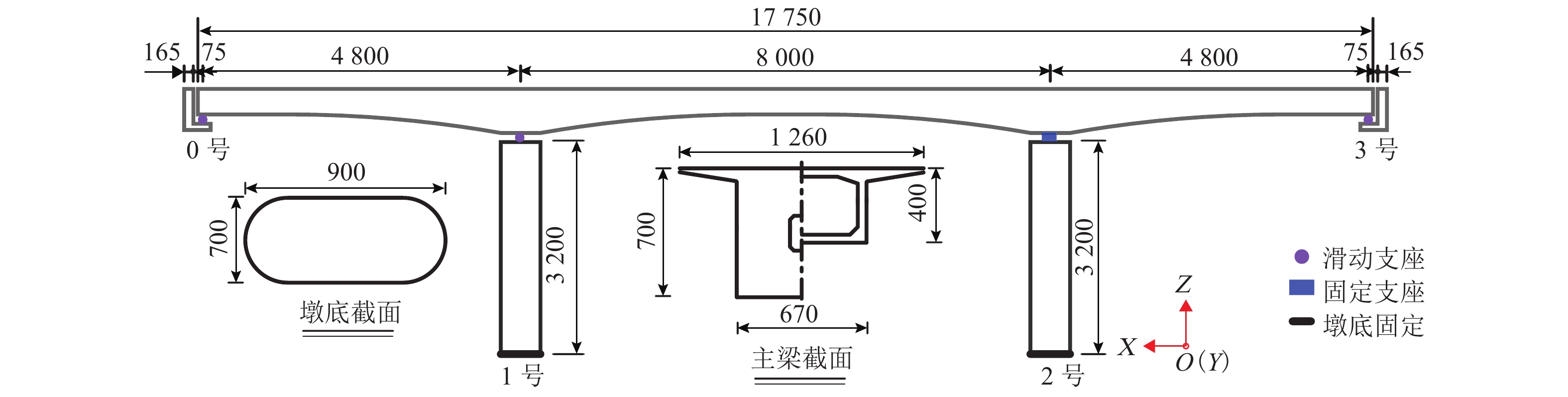
- PDF下载量: 58
- 被引次数: 0