Back-Calculation of In-situ Stress of Railway Tunnel in Xianshuihe Fault Belt
-
摘要:
地应力的分布规律严重影响地下工程建设,获得开展工程区域地应力场分布对指导支护结构的设计具有重要意义.在鲜水河断裂带某隧道工程区采用钻孔水压致裂法进行了深孔地应力测试,基于地应力测试结果,得到了开展工程区域地应力随深度的变化规律,并利用数值模拟进行地应力反演. 结果表明:地应力随深度的增加而逐渐增加,其中最大水平地应力的递增梯度为0.385 MPa/km,最小水平主应力递增梯度为0.257 MPa/km;隧道纵断面水平最大主应力为41.69 MPa,最小主应力为29.84 MPa,侧压力系数为1.363~1.438,围岩应力以水平应力为主;地应力在断层部位得到了一定程度的释放,断层两侧的完整岩体应力存在一定程度的集中,应力值较高.
Abstract:The distribution law of ground stress has a very important role in the construction of underground engineering, and obtaining the distribution of ground stress field in the construction area is of great significance to guide the design of support structure. The borehole hydraulic fracturing method was used to test the in-situ stress in the project area of a railway tunnel in Xianshuihe fault belt. Based on the in-situ stress results, the variation law of in-situ stress with depth was obtained and the in-situ stress inversion was carried out by numerical simulation. The results show that the in-situ stress increases gradually with the increase of depth, in which the increasing gradient of the maximum horizontal in-situ stress is 0.385 MPa/km, and that of the minimum horizontal principal stress is 0.257 MPa/km. The maximum and minimum horizontal principal stress values of the tunnel profile are 41.69 and 29.84 MPa, respectively. The lateral pressure coefficient is 1.363−1.438, and the surrounding rock stress is dominated by horizontal stress. Generally, the in-situ stress has been released to a certain extent in the fault area, and the stress of the intact rock mass on both sides of the fault is concentrated to a certain extent with a high stress value.
-
Key words:
- hydraulic fracturing /
- in-situ stress /
- back-calculation /
- numerical simulation
-
在科学技术和工程技术快速进步和发展的大环境下,人类致力于开发地表以下更深层的地下空间. 以往常常使用的针对地表浅层的工程类比法在深层次开挖活动中使用就不那么准确. 经过100多年的研究,国内外形成了多种地应力场模拟的方法.
朱光仪等[1-2]充分考虑岩体自重与构造应力的影响,采用三维建模结合回归分析的方法对计算区域实测地应力进行了反演. 郭运华等[3]在综合分析各种方法优劣的基础上,选择了多元回归分析法对岩体实测地应力进行反演分析. 李金锁等[4]通过原位地应力测量,确定了隧道区域的地应力状态,采用三维有限元方法确定了工程区的地应力分布. 白世伟等[5]利用不断调整侧压力系数的方法对隧道围岩地应力进行研究,表面区域的地形地貌条件以及隧道埋深都会对隧道轴线各点的地应力产生影响. 赵跃堂等[6]结合动力松弛算法和系统阻尼算法分析了大型地下结构初始地应力状态的可行性. 夏彬伟等[7]结合渝沙高速公路共和隧道地应力量测资料,获得每一组侧压系数下的测试段地应力,利用横观各向同性弹塑性本构模型计算得到隧道轴线方向的初始地应力大小和方向. 田勇等[8]利用多约束优化方法,通过目标区块边界荷载反演,进行了应力场的反演模拟. 刘宁等[9]采用非连续数值分析方法建立三维模型,利用褶皱构造和断裂构造在现今构造挤压作用下的响应方式揭示了沿线地应力存在的分区现象. 由此可见,地应力反演分析是地下工程中较为重要的环节,为设计锚固支护提供了前提条件.
本文根据对某隧道进行现场地应力测试,通过有限元模拟反演了现场围岩地应力分布规律,为岩爆和大变形预测提供依据,同时为施工隧工提供一定的指导作用.
1. 区域地质环境
1.1 地形地貌
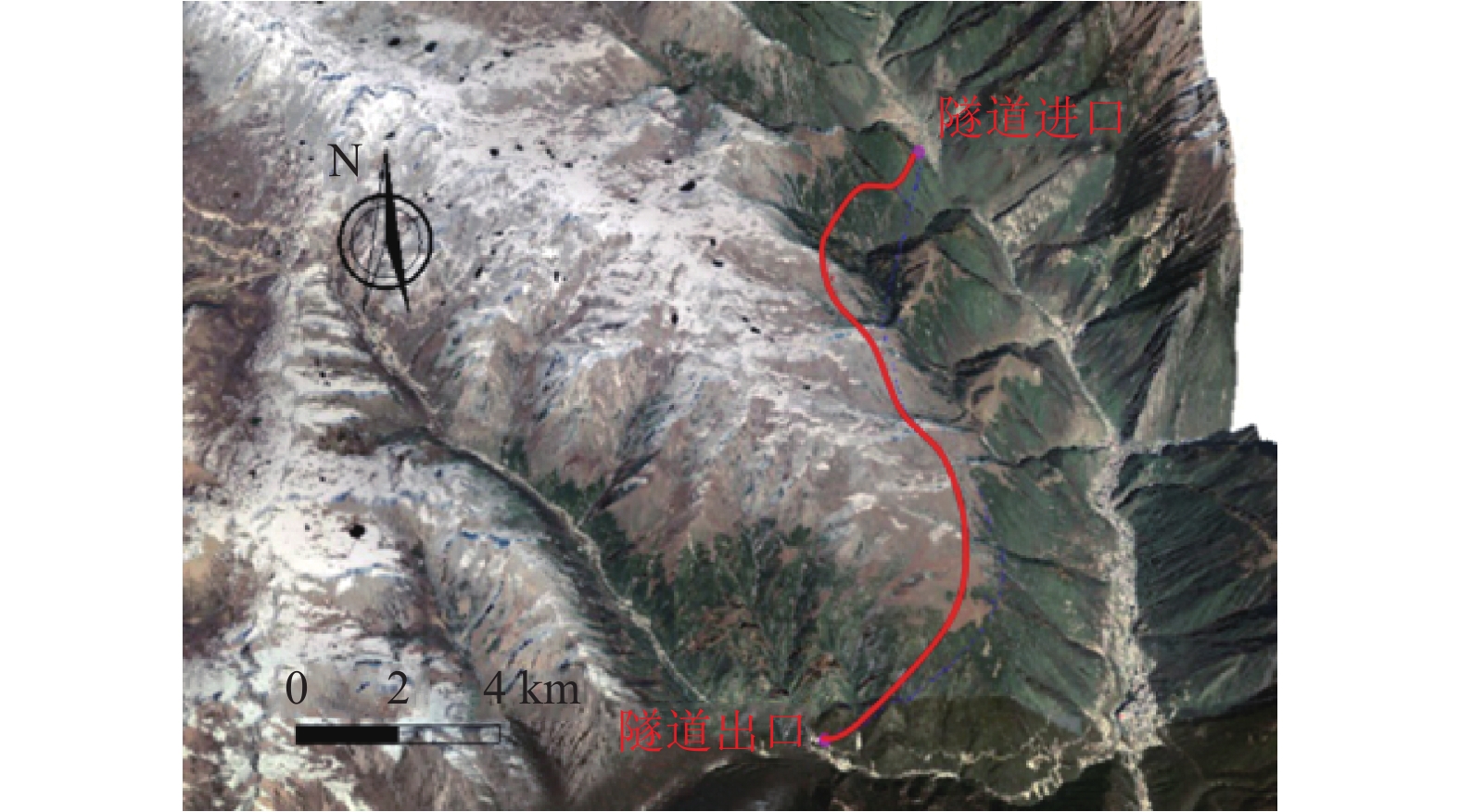
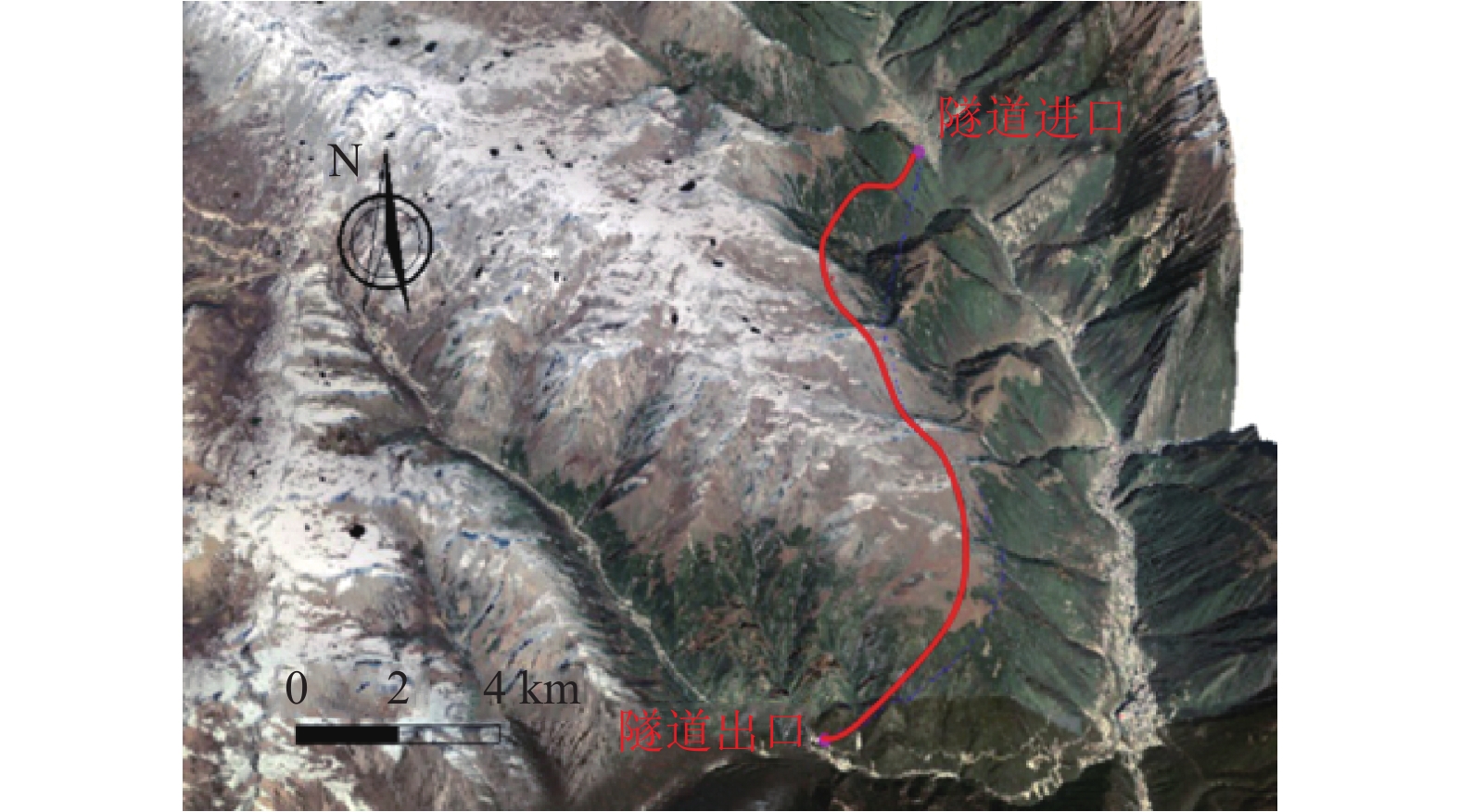
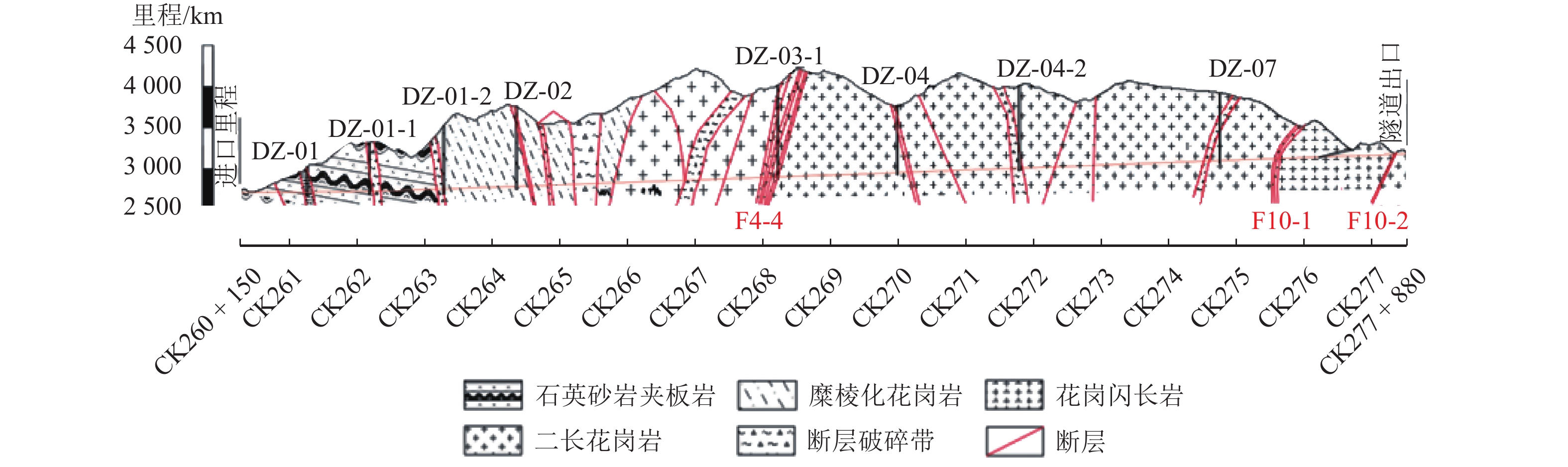
某隧道为越岭隧道,隧址区山高坡陡,属高山、极高山构造剥蚀地貌,地表高程2 715~4 225 m,外营力以冰水侵蚀作用、冻融作用为主,同时伴有生物风化等作用,为典型高原地貌. 受构造及岩性控制明显,其主体山脉走向与构造线延展方向基本一致,呈北北西展布,岭脊呈S形弯延起伏,河谷多呈V形谷. 隧址地貌如图1所示.
1.2 地层岩性
隧址区地表零星分布第四系,下伏基岩主要为侵入岩和变质岩. 变质岩主要为三叠系砂板岩,侵入岩主要为花岗闪长岩、二长花岗岩等,深大断裂附近挤压强烈变质形成糜棱岩.
1.3 地质构造
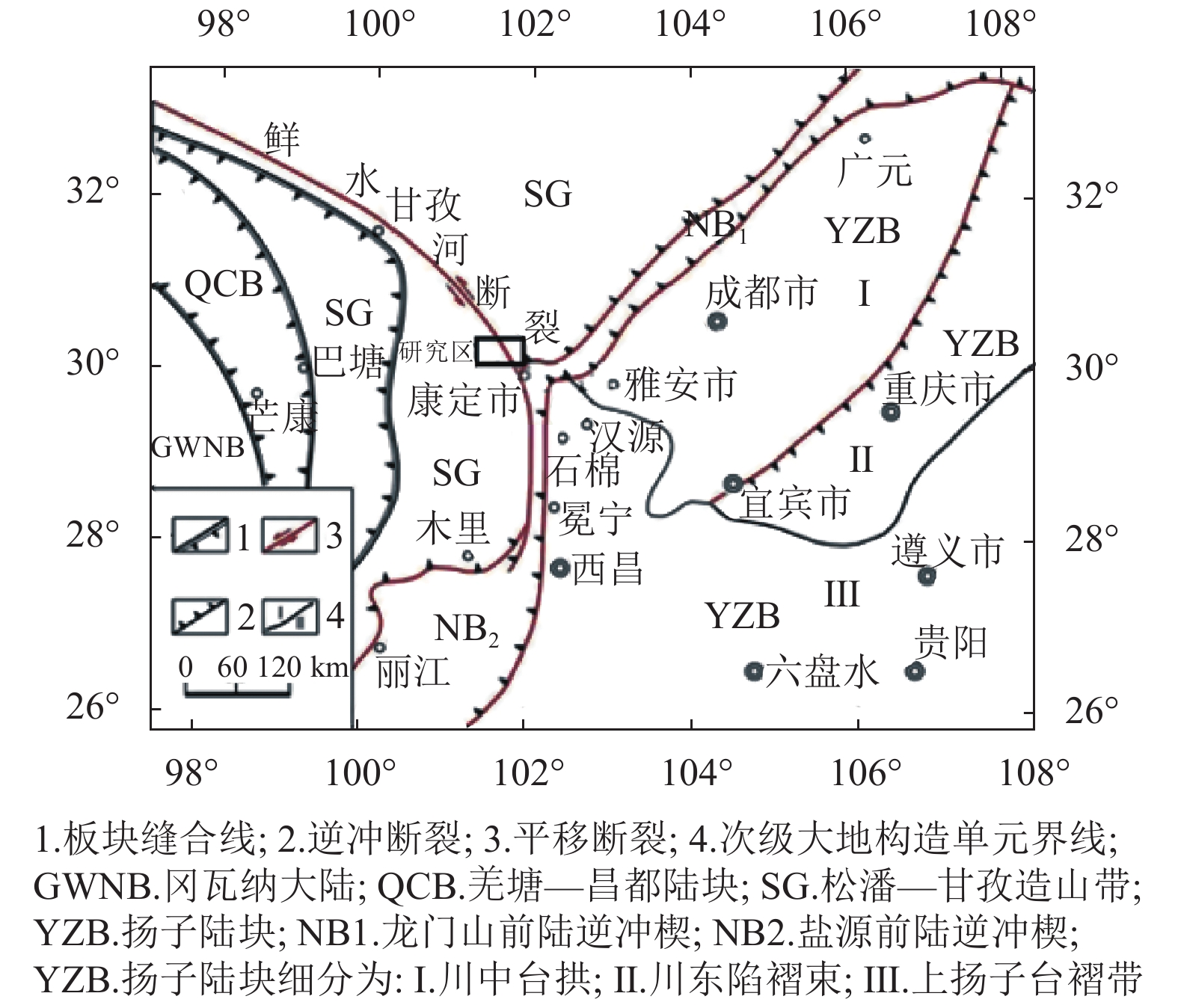
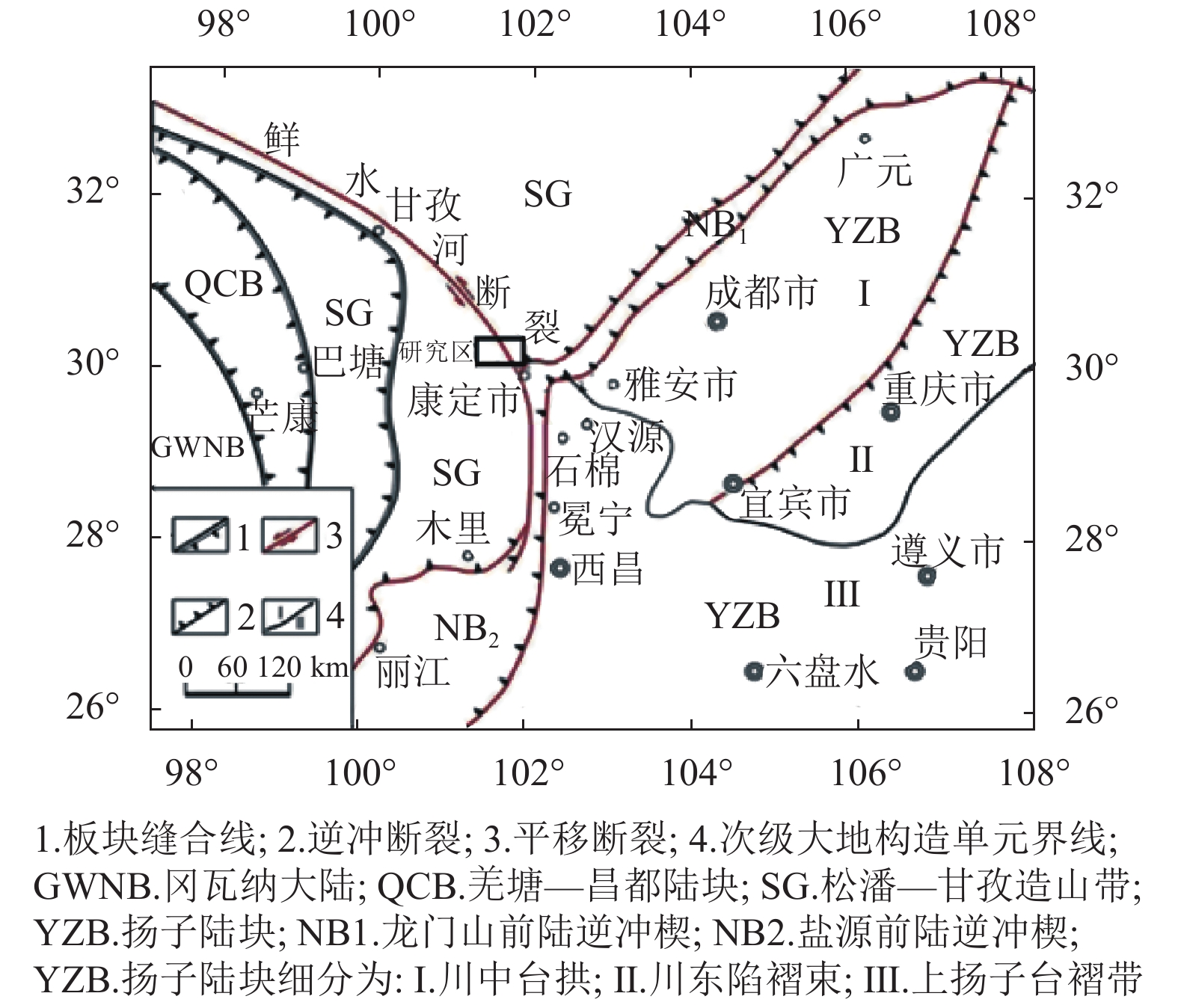
隧区在一级构造上所在区域地处扬子陆块与松潘—甘孜造山带衔接处西侧,东邻龙门山构造带,如图2所示. 区内褶皱、断裂比较发育,印支、喜山运动使盖层发生褶皱,并奠定了现今的构造轮廓,形成以北西向为主的构造线. 在二级构造上处于鲜水河构造带内,隧区主要构造形迹走向为NW向.
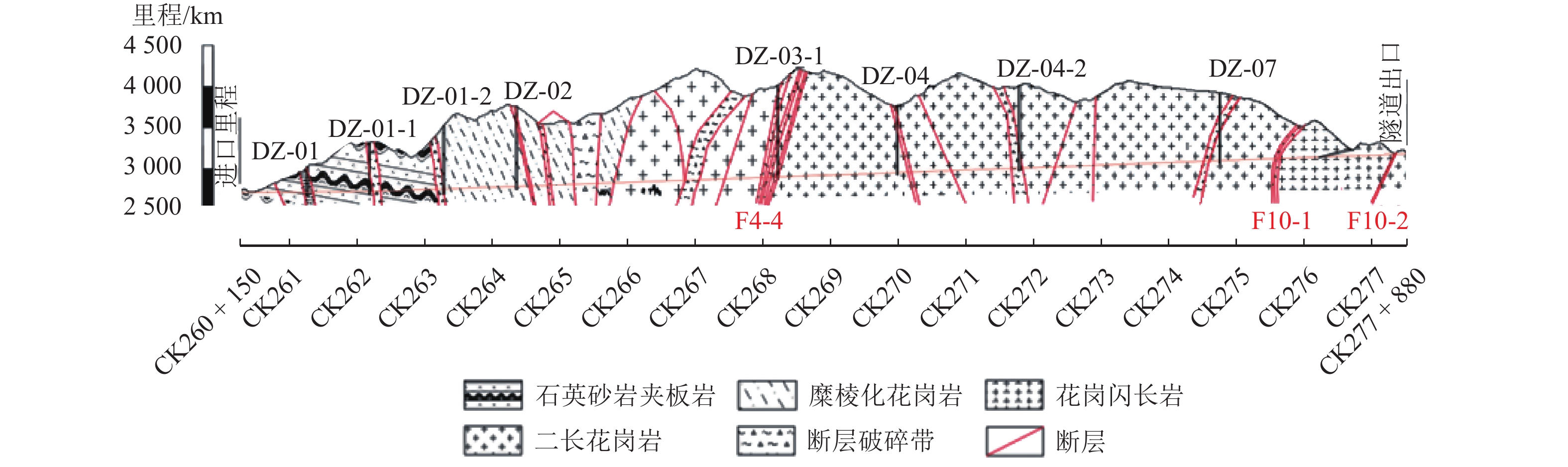
地质构造背景极为复杂,断裂发育,主要发育有鲜水河断裂分支雅拉河断裂带、色拉哈—康定断裂带、三道桥等3条区域性深大断裂以及F4-4、F10-1 、F10-2等一系列次级断裂构造,见图3 (隧道全长17730 m). 图中,DZ开头的都为测孔编号
1.4 区域地应力特征
区域应力主要来自青藏高原物质的向南东方向逃逸所产生的挤压应力,主要受鲜水河断裂带左行走滑作用控制. 隧址区现今主应力方向为NW-NWW向,主应力方向与隧址区主体构造小角度相交,隧道大致走向为NE向SW行进,区域地应力和隧道轴线呈大角度相交. 整个测区属于应力集中区域,高地应力将是深埋长大隧道面临的主要工程地质问题,硬岩可能发生岩爆,软岩和断层带可能发生大变形.
2. 现场地应力测试结果及分析
水压致裂法地应力测量是目前国际上能较好地直接进行深孔地应力测量的先进方法. 该方法无需知道岩石的力学参数就可获得地层中现今地应力的多种参量,并具有操作简便、可在任意深度进行连续或重复测试、测量速度快、测值稳定可靠等优点. 缺点是只宜用铅垂孔进行测量,且必须假定铅垂方向为一个主应力方向,不适合于地形变化大的浅部. 本项研究结合该方法优点,采用数值模拟来弥补其缺点,即两种方法相互补充、对照和印证,从而提高了研究结果的可信度.
地应力测试的目的是测量地层原位地应力的大小和方向. 通过原地应力测量,确定隧道围岩的现今地壳应力状态,即原地应力的大小和方向,小型水压致裂法地应力测量是一种能够可靠而有效测量地壳深部应力的方法. 水压致裂原地应力测量是以弹性力学为基础,通过小体积、高压的流体注入,小型压裂测试在测试层位产生一条张性裂缝并将破裂扩展到远离井筒影响范围的原始地层中.
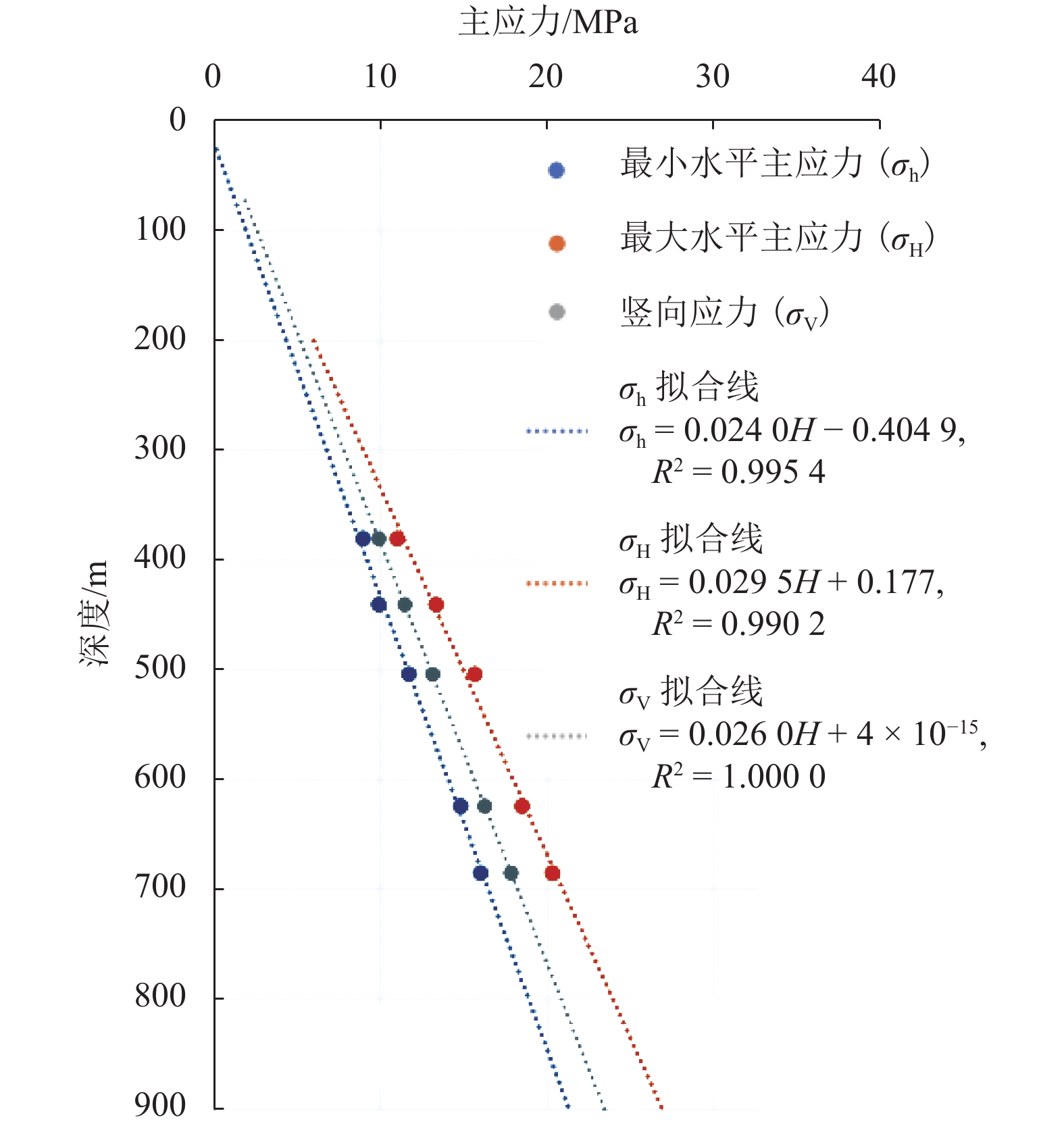
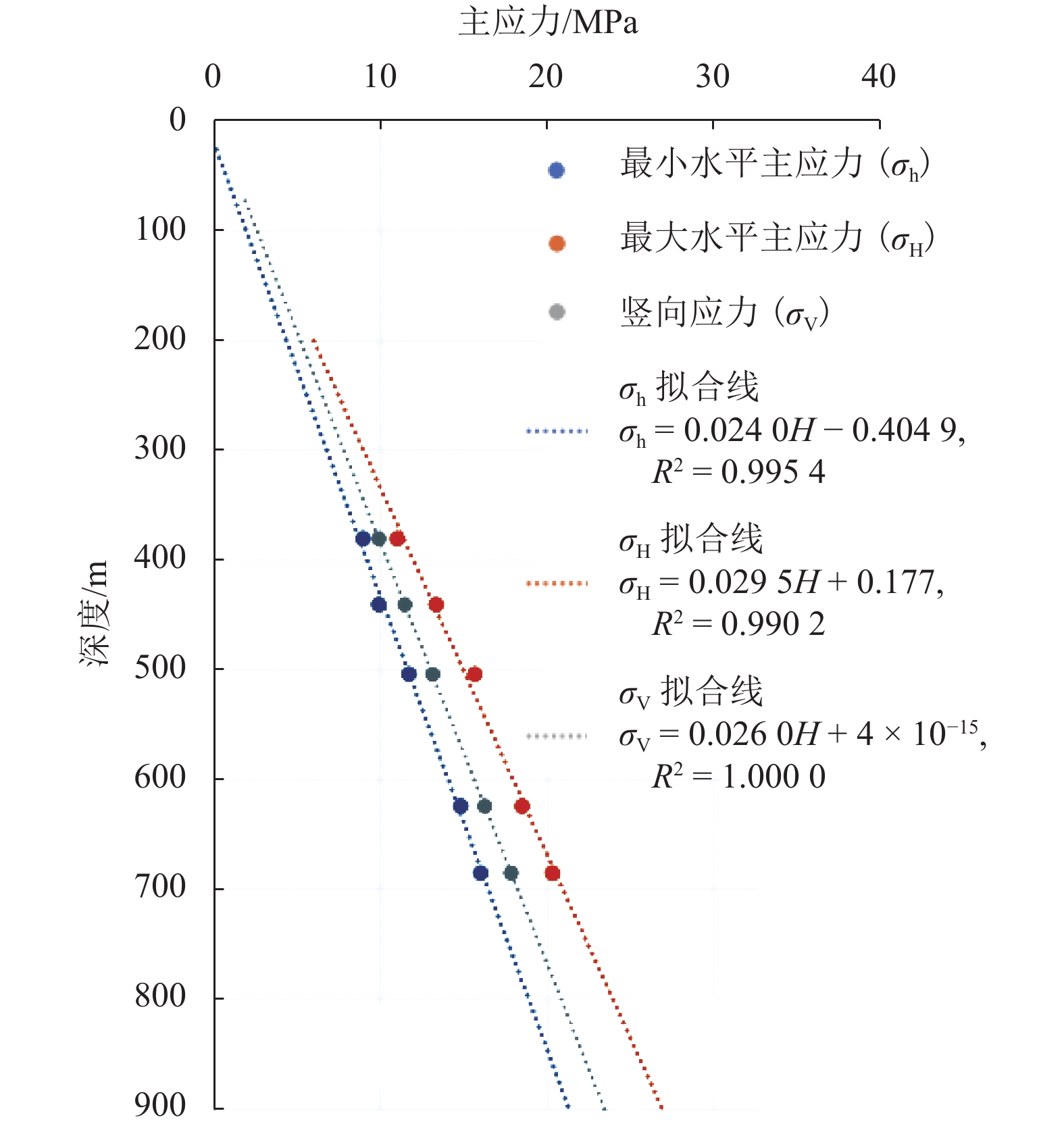
在工程区采用钻孔水压致裂法进行了深孔地应力测试,并假定:1) 测试孔是竖直的,即岩层中的竖向主应力平行于孔轴;2) 测试孔在测试段内为圆形且测试孔相对地层是无限小的,这个假定确保了弹性力学解的实用性;3) 岩层中的孔隙压力为净水压力;4) 岩石的渗透率较低,即在小型压裂过程中,测试孔内的流体压力不会在短时间内影响岩石的孔隙压力. 根据水压致裂法测试结果得到DZ-04号测孔地应力分布如图4与表1所示. 图中:H为埋深. 表1中:P0为自重压力;Pb为破裂压力;Pr为重张压力;Ps为瞬时关闭压力;以上压力值为地表监测仪器显示量值与水柱压力相加所得;T为岩石抗拉强度.
通过DZ-04号测孔应力测量结果可以分析得出:主应力值随地层深度增加而增加,在380~685 m测试深度范围内,竖向应力为9.88~17.81 MPa,最大水平主应力为10.99~20.28 MPa,最小水平主应力为8.94~15.98 MPa. 侧压系数为1.111~1.138,表明地应力以水平应力占主导,方向为N49°~N57°,和区域地应力一致.
通过拟合计算可得:最大和最小主应力的线性拟合系数分别为0.990 2和0.995 4,显示主应力随深度变化趋势的线性度较好,说明了本次水压致裂测量结果的可靠性和代表性.
表 1 DZ-04号测孔水压致裂地应力测量结果Table 1. Measurement results of ground stress caused by water pressure in borehole DZ-04测段序号 测段中心深度/m 压裂参数/MPa 应力值/MPa 破裂方向/(°) P0 Pb Pr Ps T σH σh σv 1 685.0~685.7 15.98 26.20 20.95 19.21 5.25 20.28 15.98 17.81 N53W 2 624.0~624.7 14.78 24.26 19.76 18.13 4.50 18.46 14.78 16.22 N58W 3 504.0~504.7 11.69 18.00 14.50 13.29 3.50 15.63 11.69 13.10 N65W 4 440.0~440.7 9.88 15.43 12.01 11.49 3.42 13.32 9.88 11.44 5 380.0~380.7 8.94 13.50 12.11 10.34 1.39 10.99 8.94 9.88 根据相同的方法,对其余各孔地应力分布进行线性拟合,所得结果如表2所示.
表 2 不同钻孔地应力拟合结果Table 2. In-situ stress fitting results of different boreholes孔号 拟合公式 最大值/
MPa最小值/
MPaDZ-01 σH=0.031 2H−8.027 0
σh=0.010 9H + 0.328 014.24 11.19 DZ-01-1 σH=0.022 8H−1.417 0
σh=0.009 1H + 3.226 017.88 10.84 DZ-01-2 σH=0.023 3H−0.188 4
σh=0.014 1H + 1.029 911.44 7.91 DZ-02 σH=0.042 6H−6.773 9
σh=0.031 9H−4.133 336.91 28.53 DZ-03-1 σH=0.038 9H−2.014 1
σh=0.025 4H + 1.037 841.69 29.84 DZ-04 σH=0.033 9H−0.106 7
σh=0.027 3H−0.073 827.85 22.12 DZ-04-2 σH=0.040 2H−2.167 2
σh=0.027 2H + 0.383 127.86 20.39 DZ07 σH=0.018 4H + 3.284 6
σh=0.013 4H + 1.547 318.65 13.49 3. 某隧道地应力场模拟
采用边界荷载法,施加合适的边界条件,对水压致裂法测量得到的原地应力结果进行拟合,开展隧道地应力反演,获得地应力计算结果.
3.1 模型建立
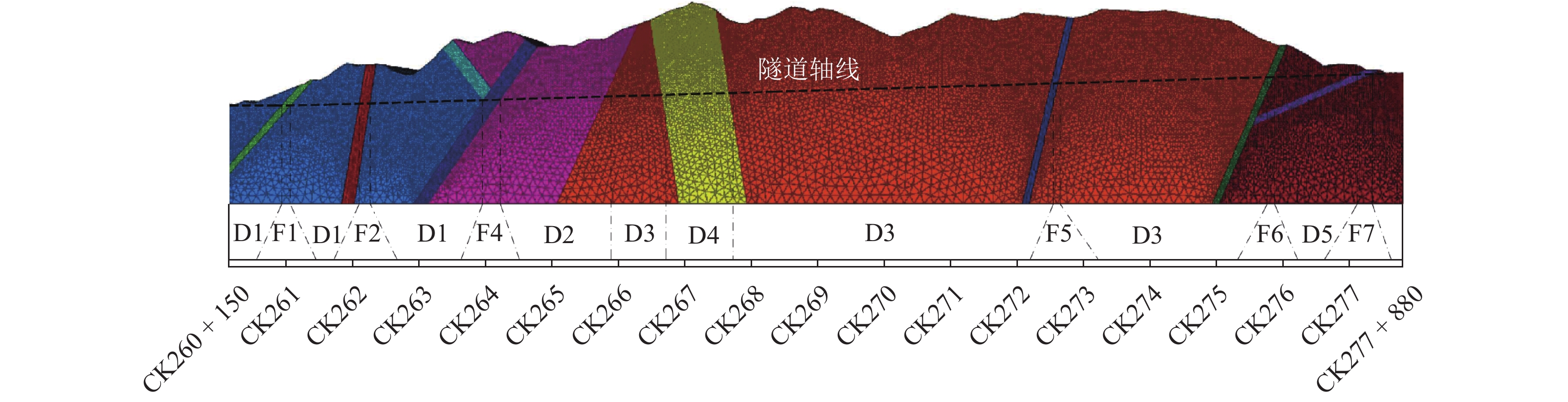
通过综合勘察绘制隧道纵断面,使用ANSYS软件建立隧道纵断面的二维模型,模型包含隧道长为17 730 m,模型厚度为1 000 m. 模型中包含隧道沿线的各个地层及断层,共有12个组和164万个网格单元,如图5所示.
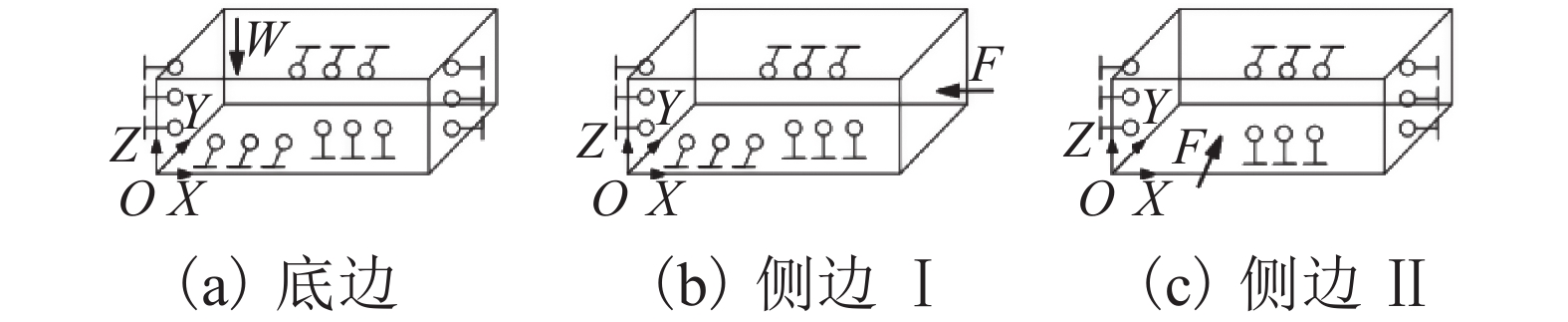
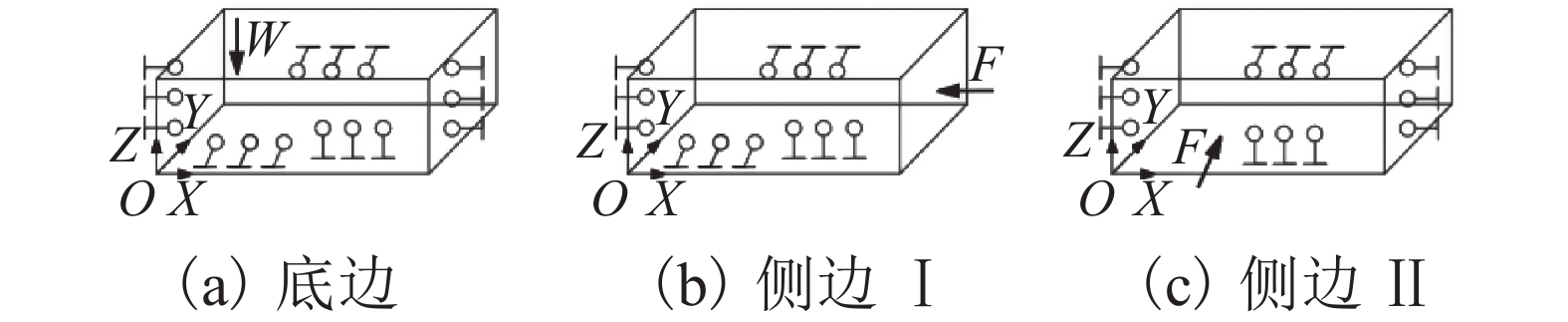
通过对隧道区域地质构造及地应力测试结果进行分析,其构造应力主要来源于NW向,因而模型的边界设置为:底边界和两个侧边界采用位移边界条件,两个侧边界采用应力边界,如图6所示. 图中:W为竖向应力;F为不同方向的水平应力.
地应力反演计算时岩体采用弹性本构模型,计算参数如表1所示. 根据不同钻孔水压致裂测得的地应力结果可以看出:隧址区的最大水平主应力在深度方向的递增梯度约为0.385 MPa/km,最小水平主应力在深度方向的递增梯度约为0.257 MPa/km. 根据隧道钻孔取样试验结果,结合《工程地质手册》(第五版)相关经验值,取隧道主要围岩物理力学参数如表3、4所示.
表 3 隧道围岩物理力学参数Table 3. Physical and mechanical parameters of tunnel surrounding rock地层 围岩
级别弹性模量/GPa 泊松比 体积模量/GPa 剪切模量/GPa 密度/
(kg•m−3)板岩 Ⅳ 20 0.32 18.52 13.20 2 630 Ⅴ 6 0.35 6.67 4.05 2 600 花岗岩 Ⅲ 50 0.25 33.33 31.25 2 750 Ⅳ 20 0.30 16.67 13.00 2 650 Ⅴ 6 0.35 6.67 4.05 2 600 糜棱岩 Ⅳ 22 0.30 18.33 14.30 2 650 Ⅴ 8 0.35 8.89 5.40 2 620 表 4 隧道断层破碎带力学参数Table 4. Mechanical parameters of tunnel fault fracture zone断层 弹性模量/
GPa泊松比 体积模量/
GPa剪切模量/
GPa密度/
(kg•m−3)F1 9.0 0.24 5.77 3.63 2 250 F2 8.5 0.25 5.67 3.40 2 250 F4 8.1 0.25 5.40 3.24 2 250 F5 8.2 0.25 5.47 3.28 2 250 F6 7.9 0.26 5.49 3.13 2 250 F7 7.5 0.26 5.21 2.98 2 250 3.2 模型验证
为验证数值反演结果的有效性,将计算结果与钻孔实测地应力进行比较,限于篇幅,仅将DZ-04与DZ-07号钻孔与数值模拟结果进行对比,如表5、6所示.
通过对比可得:数值模拟计算的结果与地应力测量结果大致相当,拟合度均接近于1,表明数值计算的拟合精度较高,其计算结果真实可信.
表 5 DZ-04应力计算结果与实测应力值对比Table 5. Comparison between calculated and measured results of dZ-04 horizontal principal stress测段序号 深度/m σH σh σv 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 1 835.7 27.85 30.8 111 22.12 21.87 99 21.71 23.45 108 2 705.7 24.23 27.56 114 20.45 19.45 95 18.33 20.22 110 3 635.7 21.27 22.35 105 16.74 17.36 104 16.51 14.53 88 4 540.7 18.80 18.56 99 14.58 15.98 110 14.04 12.56 89 5 455.7 14.78 14.32 97 12.23 13.65 112 11.83 9.76 83 表 6 DZ-07应力计算结果与实测应力值对比Table 6. Comparison between calculated and measured results of dZ-07 maximum horizontal principal stress测段序号 深度/m σH σh σv 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 1 294.5 8.91 8.45 95 5.85 6.21 106 7.80 8.32 107 2 382.5 10.39 11.20 108 7.13 8.02 112 10.14 11.23 111 3 546.6 13.03 12.67 97 9.07 10.08 111 14.48 15.64 108 4 659.5 15.06 14.76 98 10.80 11.78 109 17.48 18.56 106 5 758.5 17.75 16.87 95 12.69 13.65 108 20.10 21.47 107 6 835.0 18.65 19.19 103 13.49 15.04 111 22.13 24.51 111 3.3 结果分析
3.3.1 最大主应力
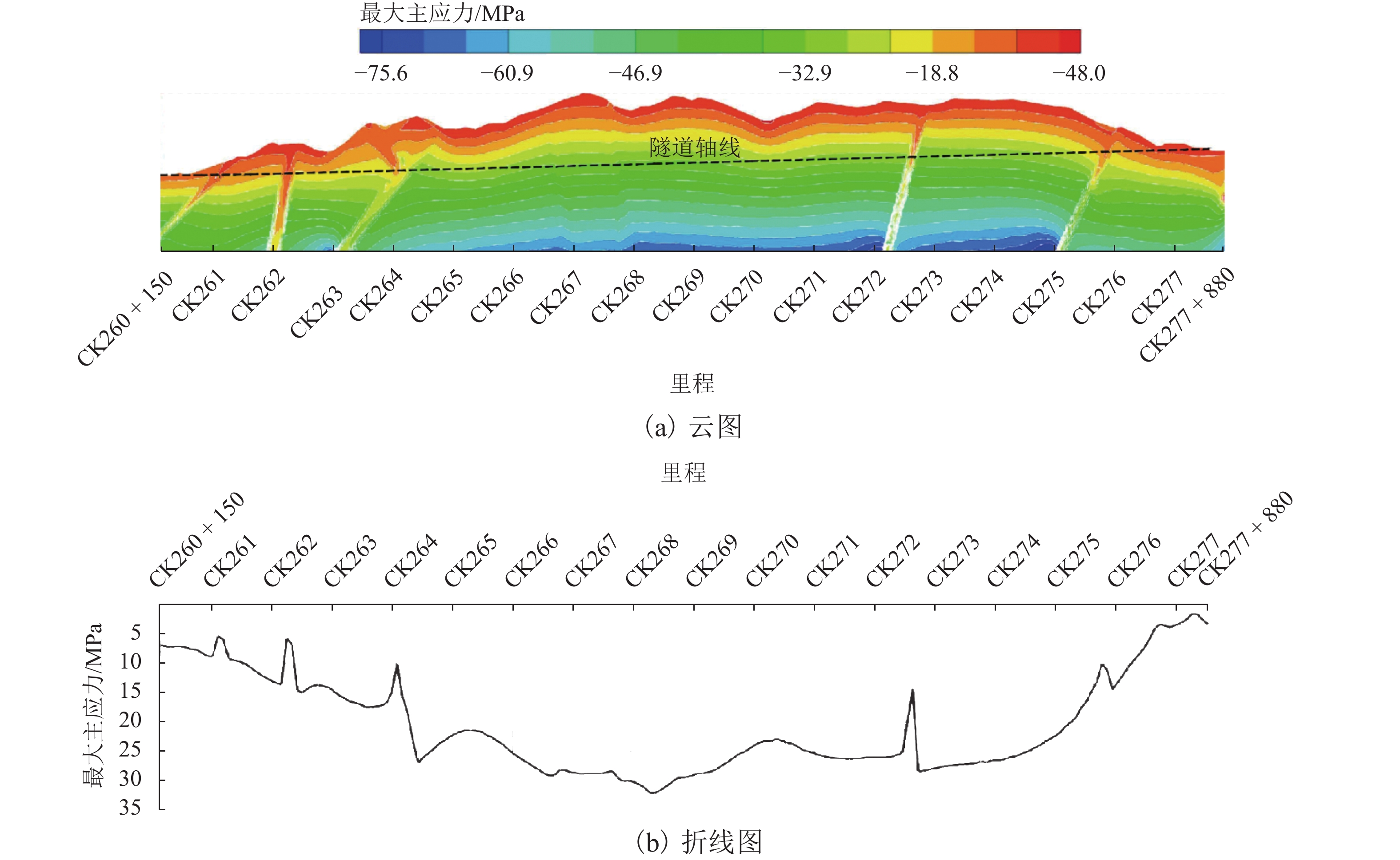
根据隧道数值模拟结果,提取模型最大主应力云图与最大主应力折线图如图7所示.
从云图及折线图可以看出,隧道沿线最大地应力随埋深、岩性、断裂构造的变化而有所变化,将其分段描述如下:
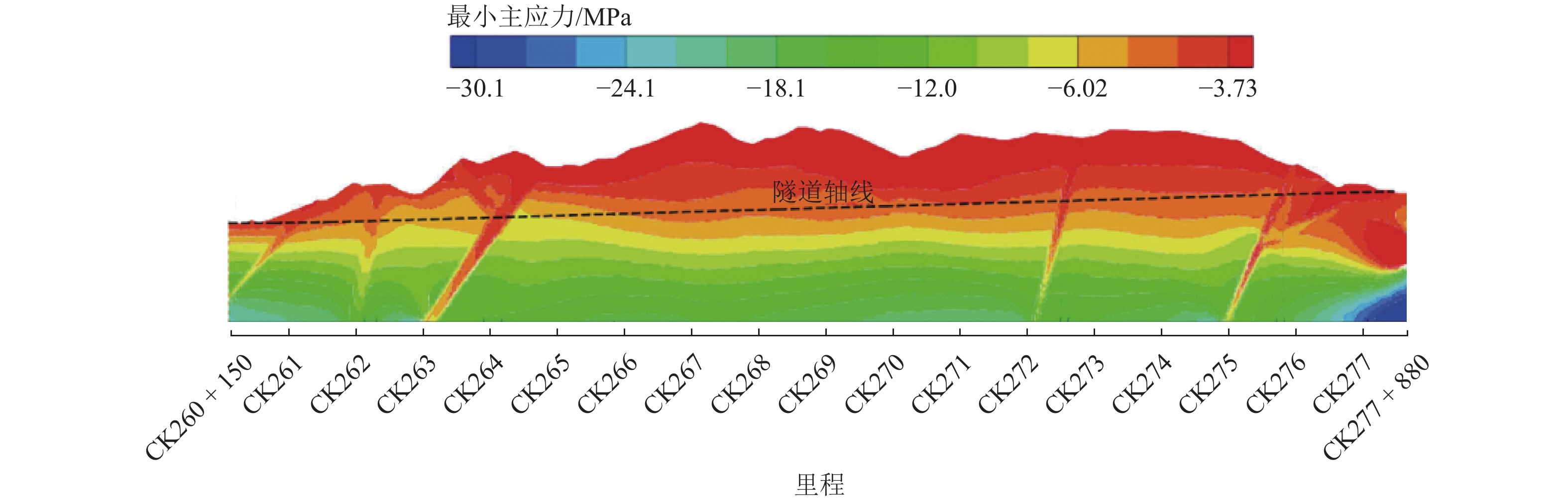
1) CK260 + 150~CK263 + 960最大主应力值为3.80~17.48 MPa,无断层的位置最大主应力为6.86~22.48 MPa,在断层F1、F2发育位置,最大主应力值则急剧减小,最小值在F1(CK261)位置,为3.80 MPa.
2) CK263 + 960~CK265 + 892最大主应力值为9.47~26.62 MPa,在断层F4位置(CK263 + 960),最大主应力值则急剧减小,为9.47 MPa.
3) 由于隧道在里程段CK265 + 892~CK272 + 488埋深最大,且其岩性为花岗闪长岩和二长花岗岩,重度较大,其最大主应力值在整个隧道轴线中是最大的,为22.94~32.14 MPa.
4)由于 CK272 + 488~CK277 + 880地形变化,隧道埋深逐渐减小,最大主应力值缓慢变小,其值为1.49~21.40 MPa,同样存在断层位置减小,断层两端增大的规律.
5) 由于在CK263 + 942处地形变化大,所以地应力也急剧增加. 在该里程段中存在5个岩性分界面,分别位于CK263 + 960、 CK265 + 892、 CK266 + 717、 CK267 + 726、CK275 + 954,在这些位置最大主应力值均有所增加.
隧道各里程段最大主应力值如表7所示:
综合最大主应力云图、折线图以及表7可以看出:最大主应力值主要受到埋深、岩石重度以及构造应力的影响,在构造应力一定的条件下,埋深越大,岩石重度越大,则最大主应力的值越大. 而在断层位置,由于岩石破裂使应力得到释放,所以最大主应力值比同深度的完整岩体较低;在岩性分界面以及断裂附近的上盘,则会发生应力集中,出现应力增高的现象.
表 7 隧道各里程段最大主应力值Table 7. Maximum principal stress in each mileage section of tunnel地层或断层编号 里程段 最大主应力
值/MPaD1 CK260 + 150~CK261 + 000 6.86~9.02 F1 CK261 + 000~CK261 + 200 3.80~7.97 D1 CK261 + 200~CK262 + 154 8.75~13.57 F2 CK262 + 154~CK262 + 405 4.53~14.33 D1 CK262 + 405~CK263 + 960 13.60~17.48 F4 CK263 + 960~CK264 + 412 9.47~27.13 D2 CK264 + 412~CK265 + 892 21.32~26.62 D3 CK265 + 892~CK266 + 717 25.80~30.15 D4 CK266 + 717~CK267 + 726 25.80~30.15 D3 CK267 + 726~CK267 + 873 25.80~30.15 D3 CK267 + 873~CK268 + 652 30.01~32.14 D3 CK268 + 652~CK272 + 488 22.94~29.83 F5 CK272 + 488~CK272 + 639 10.58~18.84 D3 CK272 + 639~CK275 + 648 13.63~21.40 F6 CK275 + 648~CK275 + 954 8.86~14.36 D5 CK275 + 954~CK277 + 880 1.49~12.81 3.3.2 中间主应力
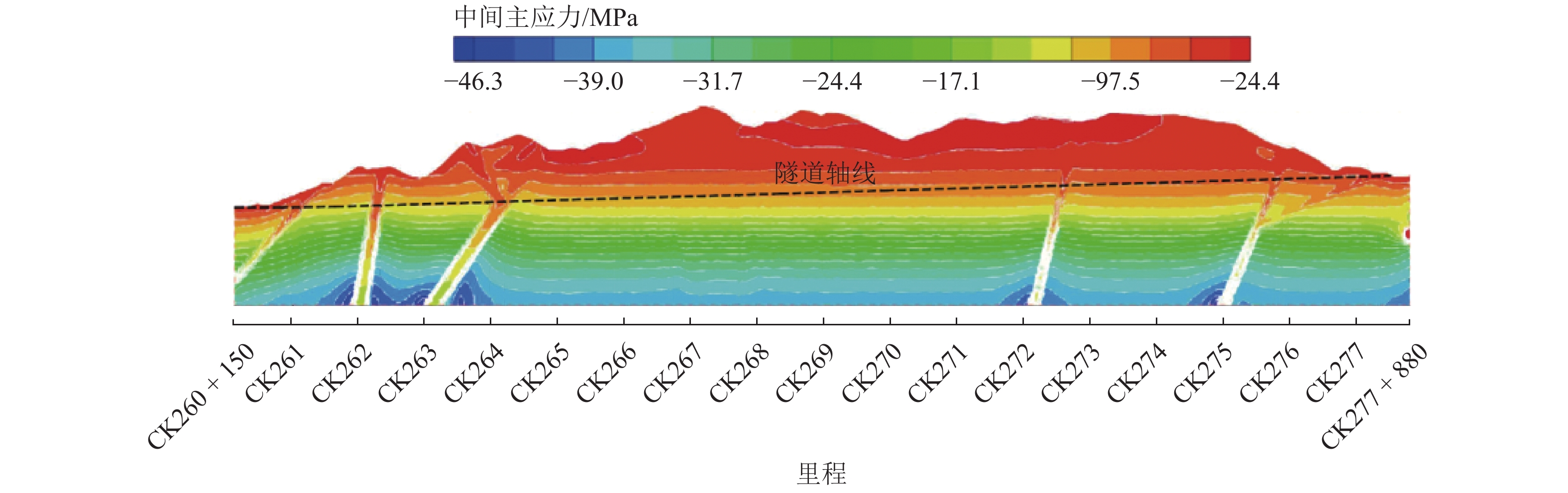
根据隧道数值模拟结果,提取模型中间主应力云图如图8所示.
由图8可知:隧道中间主应力值在0~30.21 MPa,与最大主应力有相似的规律,随埋深变化明显,在断层部位有所下降,而在断层的两端围岩中则发生了应力集中,隧道轴线沿线中间主应力值约在0~13.00 MPa.
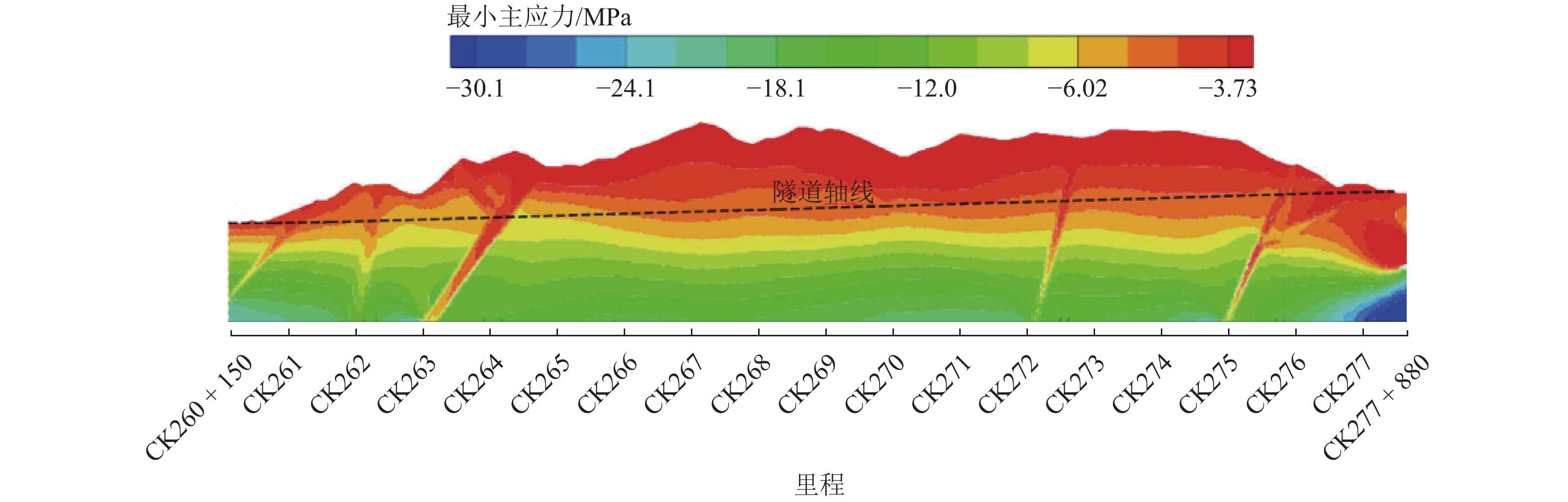
3.3.3 最小主应力
模型最小主应力云图如图9所示. 由图9可知:隧道最小主应力值在0~29.84 MPa,与最大主应力有相似的规律,随埋深变化明显,在断层部位有所下降,而在断层的两端围岩中则发生了应力集中,隧道轴线沿线最小主应力值在0~6.00 MPa.
综上所述:隧道沿线最大主应力值主要受埋深的影响,隧道埋深越大,其围岩最大主应力越大;断裂与完整岩体交汇处最大主应力差值较大,岩性分界面处则存在应力集中,隧道开挖会扰乱这些位置的应力状况,形成应力释放条件,产生岩爆.
4. 结 论
1) 本文结合水压致裂法获取8个测孔实测地应力进行了地应力反演计算,获得洞轴线各个里程段落任一点地应力值,并获取其分布规律,为岩爆和大变形合理预测、针对性设计以及综合防控奠定了基础.
2) 隧道纵断面水平最大主应力为32.14 MPa,最小主应力为29.84 MPa,侧压力系数为1.363~1.438,最大水平主应力在深度方向的递增梯度约为0.385 MPa/km,最小水平主应力在深度方向的递增梯度约为0.257 MPa/km. 实测围岩地应力方向为NW—NWW向,和区域地应力方向基本一致,围岩地应力水平高,具备发生岩爆和大变形的条件.
3) 地应力在断层部位得到了一定程度的释放,地应力较小,断层两侧的完整岩体应力存在一定程度的集中,应力值较高. 在断层段设计和施工时应充分考虑应力的释放和局部集中因素.
4) 由于岩石物理力学试验参数偏少且离散性较大,地应力反演模型参数取值不够准确,地应力计算结果可能会存在一定误差.
致谢:感谢中铁二院院控科研项目基金(KYY2019004)对本论文的支持.
-
表 1 DZ-04号测孔水压致裂地应力测量结果
Table 1. Measurement results of ground stress caused by water pressure in borehole DZ-04
测段序号 测段中心深度/m 压裂参数/MPa 应力值/MPa 破裂方向/(°) P0 Pb Pr Ps T σH σh σv 1 685.0~685.7 15.98 26.20 20.95 19.21 5.25 20.28 15.98 17.81 N53W 2 624.0~624.7 14.78 24.26 19.76 18.13 4.50 18.46 14.78 16.22 N58W 3 504.0~504.7 11.69 18.00 14.50 13.29 3.50 15.63 11.69 13.10 N65W 4 440.0~440.7 9.88 15.43 12.01 11.49 3.42 13.32 9.88 11.44 5 380.0~380.7 8.94 13.50 12.11 10.34 1.39 10.99 8.94 9.88 表 2 不同钻孔地应力拟合结果
Table 2. In-situ stress fitting results of different boreholes
孔号 拟合公式 最大值/
MPa最小值/
MPaDZ-01 σH=0.031 2H−8.027 0
σh=0.010 9H + 0.328 014.24 11.19 DZ-01-1 σH=0.022 8H−1.417 0
σh=0.009 1H + 3.226 017.88 10.84 DZ-01-2 σH=0.023 3H−0.188 4
σh=0.014 1H + 1.029 911.44 7.91 DZ-02 σH=0.042 6H−6.773 9
σh=0.031 9H−4.133 336.91 28.53 DZ-03-1 σH=0.038 9H−2.014 1
σh=0.025 4H + 1.037 841.69 29.84 DZ-04 σH=0.033 9H−0.106 7
σh=0.027 3H−0.073 827.85 22.12 DZ-04-2 σH=0.040 2H−2.167 2
σh=0.027 2H + 0.383 127.86 20.39 DZ07 σH=0.018 4H + 3.284 6
σh=0.013 4H + 1.547 318.65 13.49 表 3 隧道围岩物理力学参数
Table 3. Physical and mechanical parameters of tunnel surrounding rock
地层 围岩
级别弹性模量/GPa 泊松比 体积模量/GPa 剪切模量/GPa 密度/
(kg•m−3)板岩 Ⅳ 20 0.32 18.52 13.20 2 630 Ⅴ 6 0.35 6.67 4.05 2 600 花岗岩 Ⅲ 50 0.25 33.33 31.25 2 750 Ⅳ 20 0.30 16.67 13.00 2 650 Ⅴ 6 0.35 6.67 4.05 2 600 糜棱岩 Ⅳ 22 0.30 18.33 14.30 2 650 Ⅴ 8 0.35 8.89 5.40 2 620 表 4 隧道断层破碎带力学参数
Table 4. Mechanical parameters of tunnel fault fracture zone
断层 弹性模量/
GPa泊松比 体积模量/
GPa剪切模量/
GPa密度/
(kg•m−3)F1 9.0 0.24 5.77 3.63 2 250 F2 8.5 0.25 5.67 3.40 2 250 F4 8.1 0.25 5.40 3.24 2 250 F5 8.2 0.25 5.47 3.28 2 250 F6 7.9 0.26 5.49 3.13 2 250 F7 7.5 0.26 5.21 2.98 2 250 表 5 DZ-04应力计算结果与实测应力值对比
Table 5. Comparison between calculated and measured results of dZ-04 horizontal principal stress
测段序号 深度/m σH σh σv 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 1 835.7 27.85 30.8 111 22.12 21.87 99 21.71 23.45 108 2 705.7 24.23 27.56 114 20.45 19.45 95 18.33 20.22 110 3 635.7 21.27 22.35 105 16.74 17.36 104 16.51 14.53 88 4 540.7 18.80 18.56 99 14.58 15.98 110 14.04 12.56 89 5 455.7 14.78 14.32 97 12.23 13.65 112 11.83 9.76 83 表 6 DZ-07应力计算结果与实测应力值对比
Table 6. Comparison between calculated and measured results of dZ-07 maximum horizontal principal stress
测段序号 深度/m σH σh σv 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 实测/MPa 计算/MPa 拟合度/% 1 294.5 8.91 8.45 95 5.85 6.21 106 7.80 8.32 107 2 382.5 10.39 11.20 108 7.13 8.02 112 10.14 11.23 111 3 546.6 13.03 12.67 97 9.07 10.08 111 14.48 15.64 108 4 659.5 15.06 14.76 98 10.80 11.78 109 17.48 18.56 106 5 758.5 17.75 16.87 95 12.69 13.65 108 20.10 21.47 107 6 835.0 18.65 19.19 103 13.49 15.04 111 22.13 24.51 111 表 7 隧道各里程段最大主应力值
Table 7. Maximum principal stress in each mileage section of tunnel
地层或断层编号 里程段 最大主应力
值/MPaD1 CK260 + 150~CK261 + 000 6.86~9.02 F1 CK261 + 000~CK261 + 200 3.80~7.97 D1 CK261 + 200~CK262 + 154 8.75~13.57 F2 CK262 + 154~CK262 + 405 4.53~14.33 D1 CK262 + 405~CK263 + 960 13.60~17.48 F4 CK263 + 960~CK264 + 412 9.47~27.13 D2 CK264 + 412~CK265 + 892 21.32~26.62 D3 CK265 + 892~CK266 + 717 25.80~30.15 D4 CK266 + 717~CK267 + 726 25.80~30.15 D3 CK267 + 726~CK267 + 873 25.80~30.15 D3 CK267 + 873~CK268 + 652 30.01~32.14 D3 CK268 + 652~CK272 + 488 22.94~29.83 F5 CK272 + 488~CK272 + 639 10.58~18.84 D3 CK272 + 639~CK275 + 648 13.63~21.40 F6 CK275 + 648~CK275 + 954 8.86~14.36 D5 CK275 + 954~CK277 + 880 1.49~12.81 -
[1] 朱光仪,郭小红,陈卫忠,等. 雪峰山公路隧道地应力场反演及工程应用[J]. 中南公路工程,2006,31(1): 71-75.ZHU Guangyi, GUO Xiaohong, CHEN Weizhong, et al. Inversion of in situ Stress and its application in xuefengshan roadway tunnel[J]. Gentral South Highway Engineering, 2006, 31(1): 71-75. [2] 邱祥波,李术才,李树忱. 三维地应力回归分析方法与工程应用[J]. 岩石力学与工程学报,2003(10): 1613-1617. doi: 10.3321/j.issn:1000-6915.2003.10.007QIU Xiangbo, LI Shucai, LI Shuchen, et al. 3D Geostress regression analysis method and its application[J]. Chinese Journal of Rock Mechanics and Engineering, 2003(10): 1613-1617. doi: 10.3321/j.issn:1000-6915.2003.10.007 [3] 郭运华,朱维申,李新平,等. 基于FLAC3D改进的初始地应力场回归方法[J]. 岩土工程学报,2014,36(5): 892-898. doi: 10.11779/CJGE201405012GUO Yunhua, ZHU Weishen, LI Xinping, et al. Improved regression method for initial geostress based on FLAC3D[J]. Chinese Journal of Geotechnical Engineering, 2014, 36(5): 892-898. doi: 10.11779/CJGE201405012 [4] 李金锁,彭华,马秀敏,等. 大丽线铁路隧道工程地应力三维有限元数值模拟分析[J]. 岩土工程学报,2006,28(6): 800-803. doi: 10.3321/j.issn:1000-4548.2006.06.025LI Jinsuo, PENG Hua, MA Xiumin, et al. Three-dimensional finite element numerical simulation of geo-stress in Da-Li Railway tunnel of Yunnan[J]. Chinese Journal of Geotechnical Engineering, 2006, 28(6): 800-803. doi: 10.3321/j.issn:1000-4548.2006.06.025 [5] 白世伟,韩昌瑞,顾义磊,等. 隧道应力扰动区地应力测试及反演研究[J]. 岩土力学,2008,29(11): 2887-2891. doi: 10.3969/j.issn.1000-7598.2008.11.001BAI Shiwei, HAN Changrui, GU Yilei, et al. Researth on crustral strese measurement and inversion of stress disturbed area of a tunnel[J]. Rock and Soil Mechanics, 2008, 29(11): 2887-2891. doi: 10.3969/j.issn.1000-7598.2008.11.001 [6] 赵跃堂,范斌,梁晖,等. 大型深埋隧道初始地应力状态的确定[J]. 地下空间与工程学报,2008,4(1): 147-151.ZHAO Yuetang, FAN Bin, LIANG Hui, et al. Determination of initial earth stress of large deeply-buried tunnel[J]. Chinese Journal of Underground Space and Engineering, 2008, 4(1): 147-151. [7] 夏彬伟,李晓红,韩昌瑞,等. 深埋隧道层状岩体地应力反演研究[J]. 水文地质工程地质,2009,36(4): 75-79. doi: 10.3969/j.issn.1000-3665.2009.04.017XIA Binwei, LI Xiaohong, HAN CHangrui, et al. Study on inversion of in-situ stress of layered rockmass in buried tunnel[J]. Hydrogeology & Engineering Geology, 2009, 36(4): 75-79. doi: 10.3969/j.issn.1000-3665.2009.04.017 [8] 田勇,俞然刚,张能,等. 基于ANSYS软件地应力反演的数值实验技术[J]. 实验技术与管理,2019,36(2): 168-170.TIAN Yong, YU Rangang, ZHANG Neng, et al. Numerical experimental technology for geo-stress inversion based on ANSYS software[J]. Experimental Technology and Management, 2019, 36(2): 168-170. [9] 刘宁,张春生,褚卫江,等. 超深埋长隧道地应力场综合反分析方法与应用[J]. 中国公路学报,2018,31(10): 69-78. doi: 10.3969/j.issn.1001-7372.2018.10.007LIU Ning, ZHANG Chunsheng, CHU Weijiang, et al. Comprehensive inversion analysis method and application of deep buried long tunnel geo-stress field[J]. China Journal of Highway and Transport, 2018, 31(10): 69-78. doi: 10.3969/j.issn.1001-7372.2018.10.007 期刊类型引用(6)
1. 耿招,黄纲领,雷安平. 深埋特长隧道地应力反演与围岩稳定性分析. 路基工程. 2025(03): 225-231 .  百度学术
百度学术2. 李剑,李旋,颜良宇,王潇,周亮. 长大深埋隧道三维地应力场多元回归反演分析. 交通科技. 2024(03): 105-109 .  百度学术
百度学术3. 冯涛,蒋良文,袁东,林之恒,孟少伟,陈宇. 鲜水河构造带隧道高地应力区岩爆特性分析. 铁道工程学报. 2024(08): 74-78+85 .  百度学术
百度学术4. 刘杰,陈志峰,高红星,张进鹏,马明,吴星辉,方庆河,张琰岽,吴继飞. 滕县煤田下组煤地应力特征与灰岩夹层顶板稳定性控制研究. 山东科技大学学报(自然科学版). 2024(06): 10-23 .  百度学术
百度学术5. 李金轩,郭松峰,祁生文,张亚国,郑博文,唐凤娇,马行东,张世殊. 青藏高原东缘应力场及地下工程灾害风险研究. 工程地质学报. 2023(03): 736-749 .  百度学术
百度学术6. 任洋,吴岳华,李天斌. 深埋长隧道纵剖面初始地应力插值计算方法及应用. 隧道建设(中英文). 2023(11): 1908-1915 .  百度学术
百度学术其他类型引用(2)
-






 下载:
下载:








 下载:
下载:
 百度学术
百度学术