桥梁柔性对中低速磁浮车辆平曲线通过的影响

李苗; 尚贤洪; 李铁; 陈晓昊; 罗世辉; 马卫华; 雷成

doi:10.3969/j.issn.0258-2724.20210872

桥梁柔性对中低速磁浮车辆平曲线通过的影响

doi: 10.3969/j.issn.0258-2724.20210872

李苗^1,,
尚贤洪¹,
李铁^{1, 2},
陈晓昊¹,
罗世辉¹,
马卫华^1, ,,
雷成³

1.
西南交通大学牵引动力国家重点实验室，四川成都 610031
2.
中车大连机车车辆有限公司，辽宁大连 116022
3.
郑州铁路职业技术学院河南省轨道交通智能安全工程技术研究中心，河南郑州 451460

基金项目: 国家自然科学基金（51875483，52102442）

详细信息

作者简介:
李苗（1991—），男，博士研究生，研究方向为常导磁浮车辆系统动力学，E-mail：limiao_0915@hotmail.com

通讯作者:
马卫华（1979—），男，研究员，研究方向为磁浮列车悬浮架设计、磁浮列车系统动力学，E-mail：mwh@swjtu.edu.cn

中图分类号: U237
计量
- 文章访问数: 297
- HTML全文浏览量: 119
- PDF下载量: 14
- 被引次数: 0
出版历程
- 收稿日期: 2021-11-08
- 修回日期: 2022-02-07
- 刊出日期: 2022-03-05

Influence of Bridge Flexibility on Horizontal Curve Passing of Medium-Low-Speed Maglev Vehicles

LI Miao^1
,,
SHANG Xianhong¹,
LI Tie^{1, 2},
CHEN Xiaohao¹,
LUO Shihui¹,
MA Weihua^{1
, ,},
LEI Cheng³

1.
State Key Laboratory of Traction Power, Southwest Jiaotong University, Chengdu 610031, China
2.
CRRC Dalian Locomotive and Rolling Stock Co., Ltd., Dalian 116022, China
3.
Henan Engineering Research Center of Rail Transit Intelligent Security, Zhengzhou Railway Vocational & Technical College, Zhengzhou 451460, China

摘要

摘要:
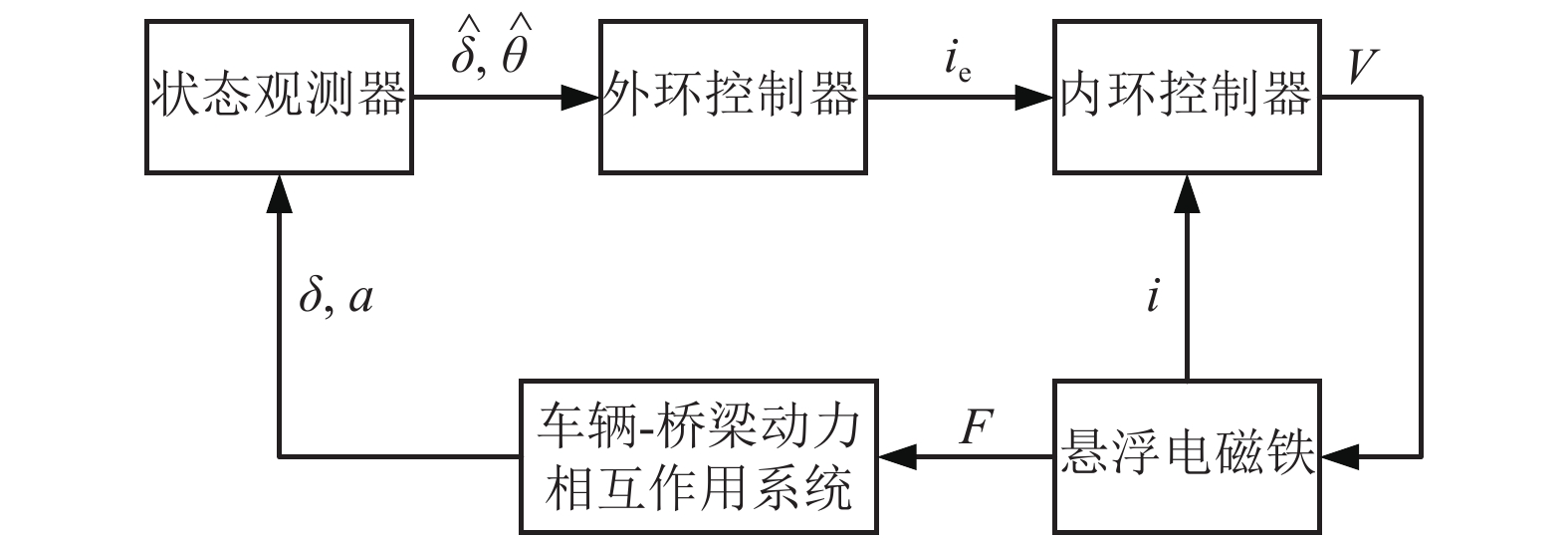
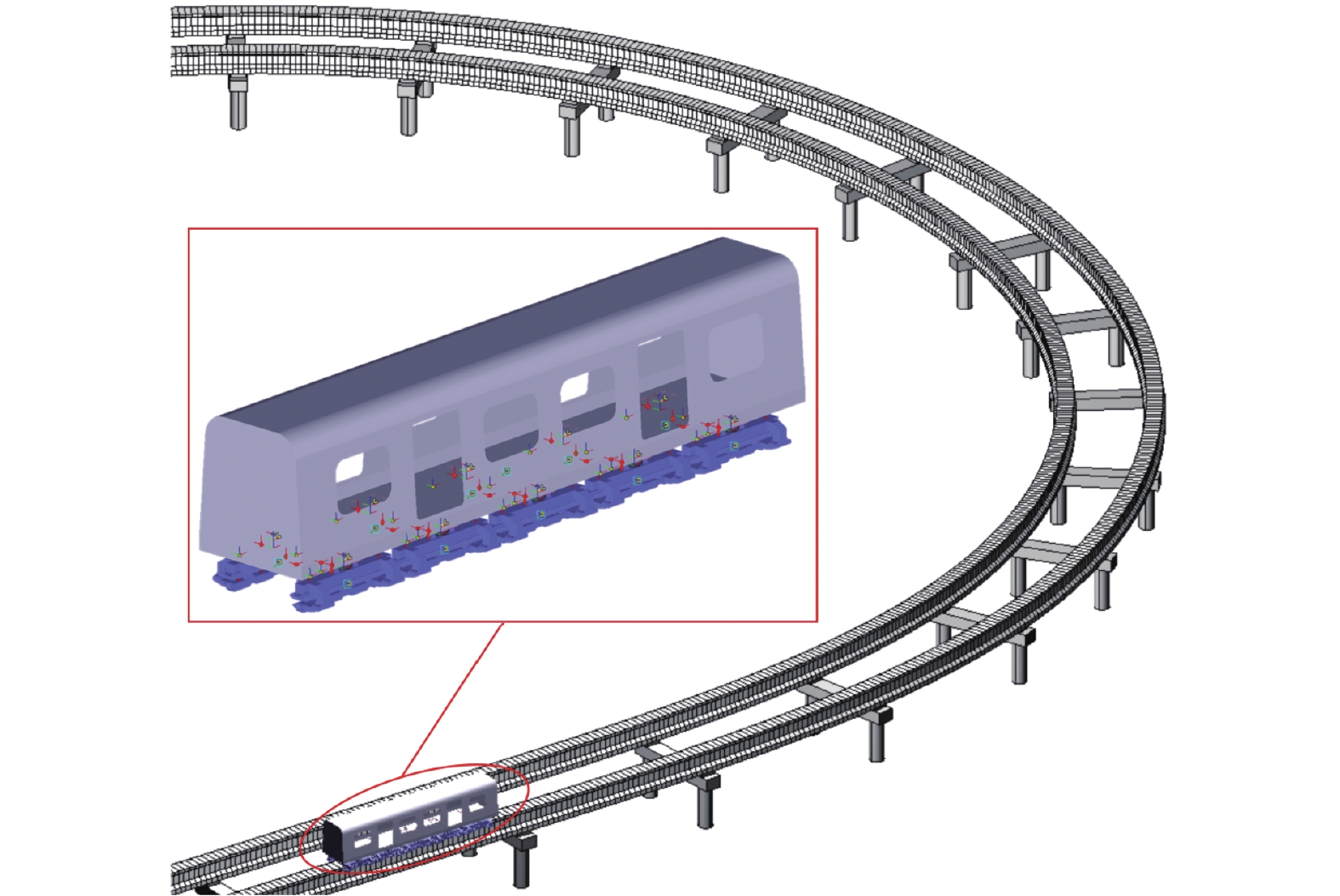
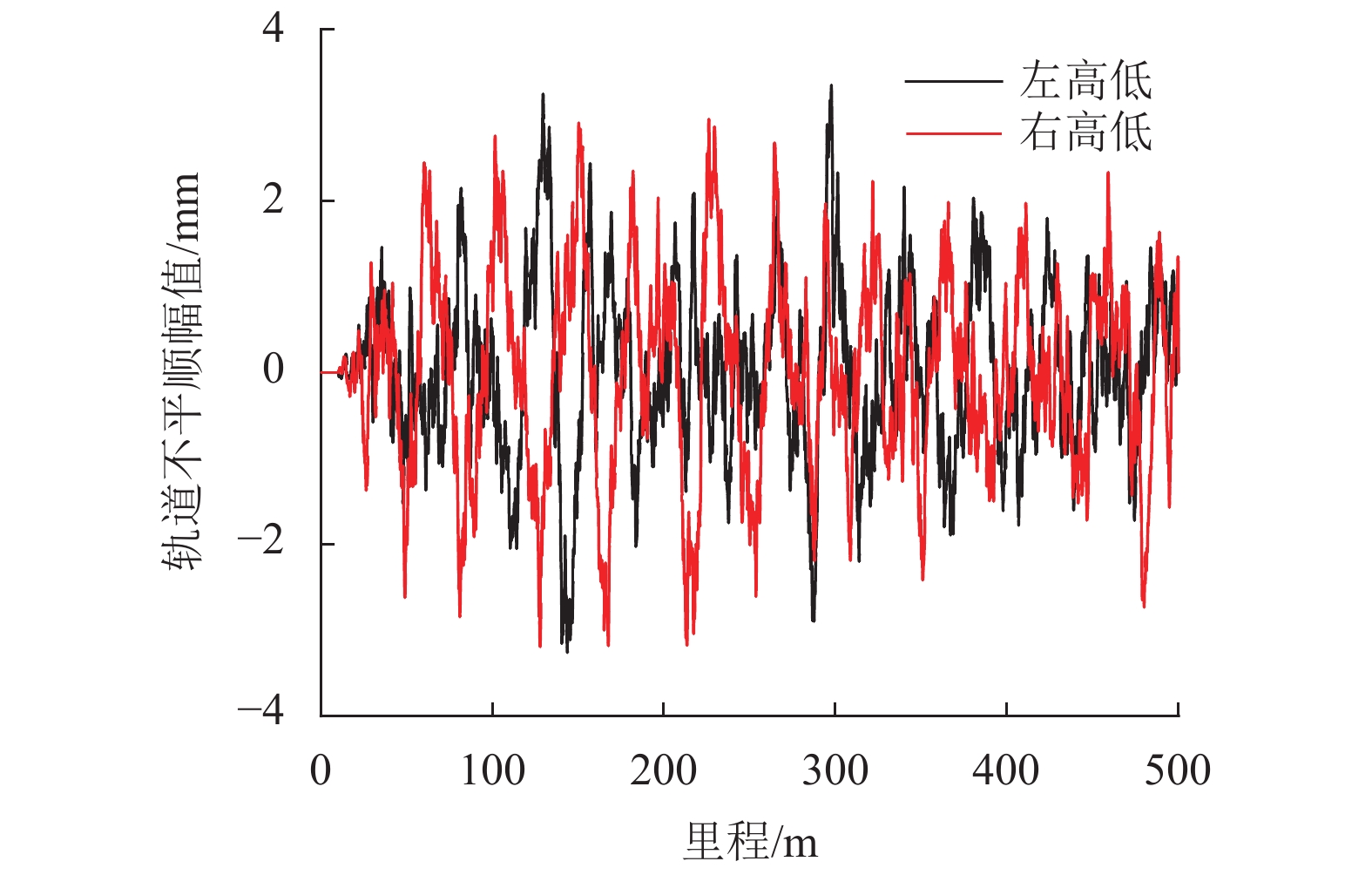
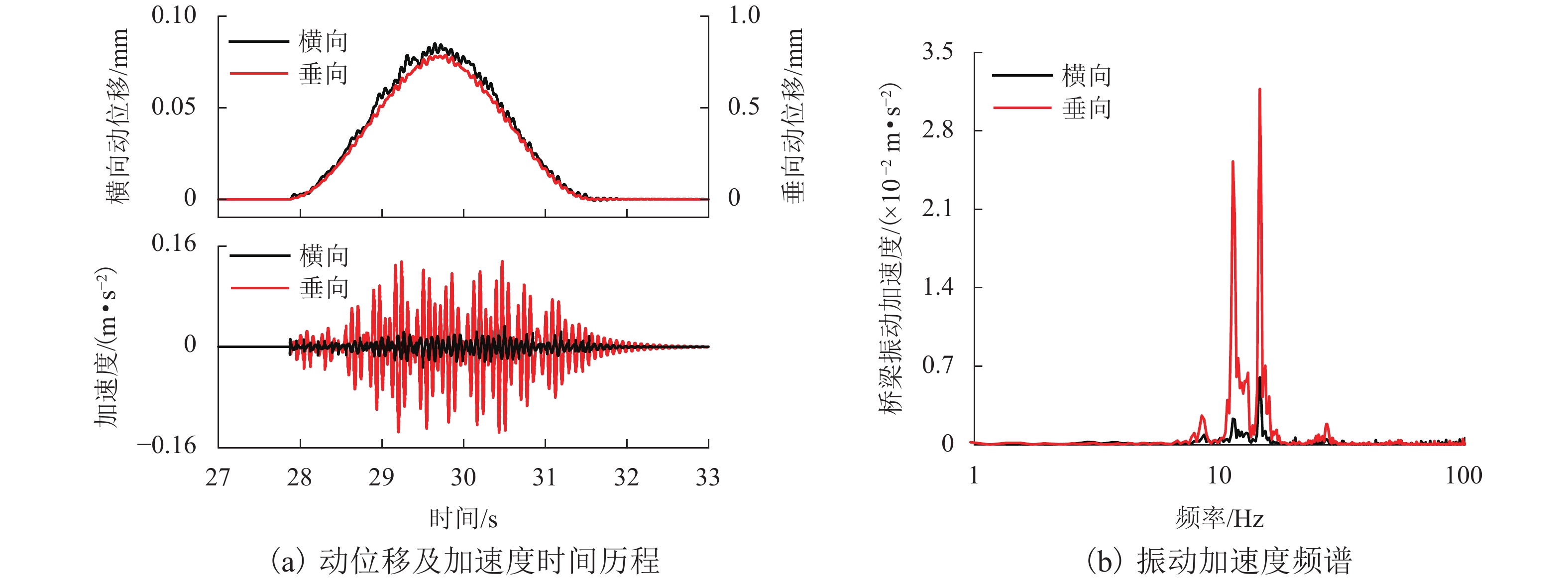
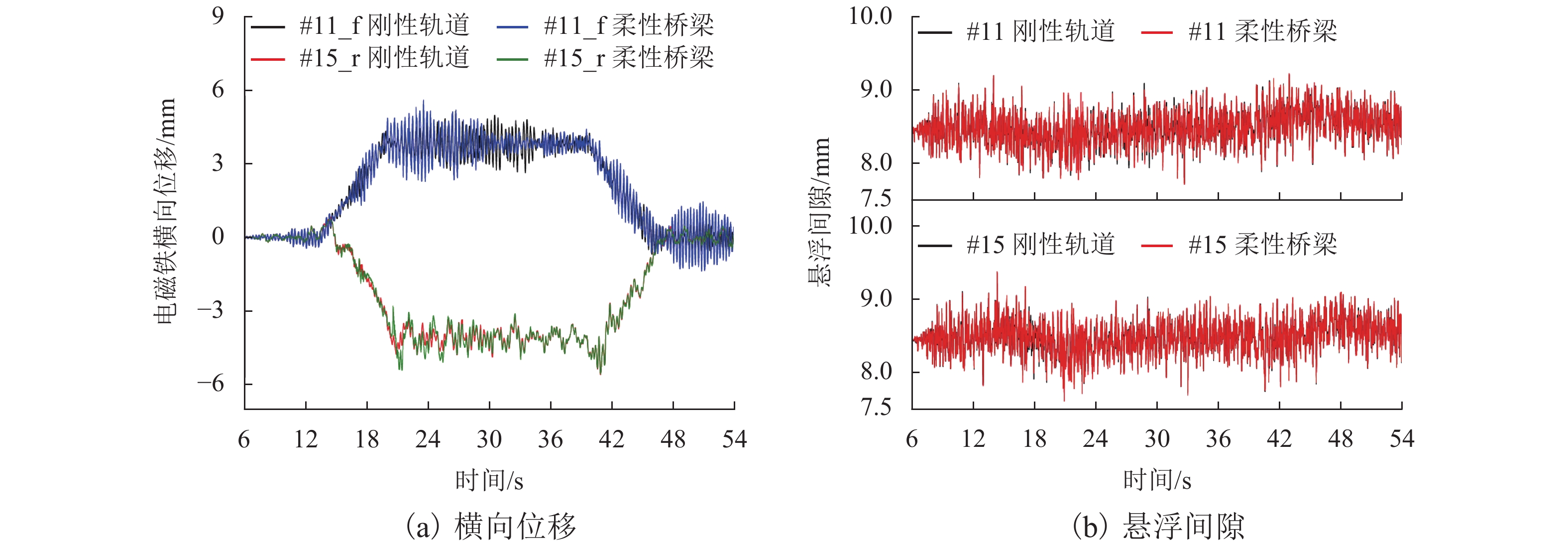
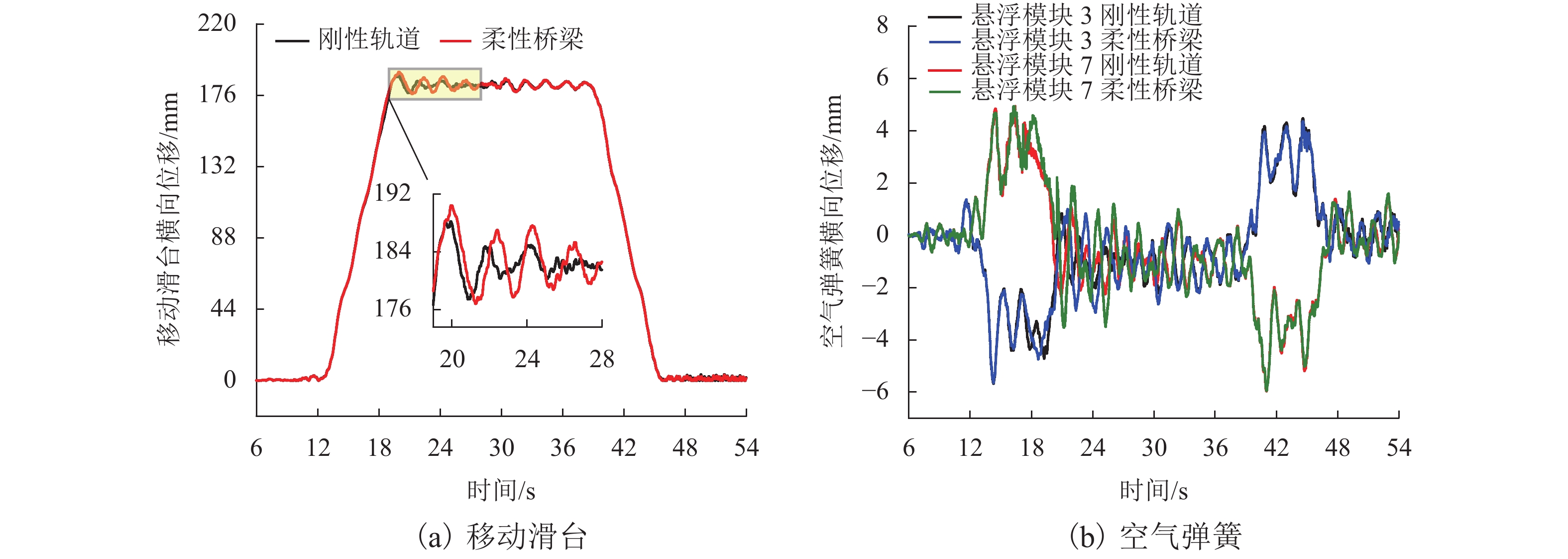
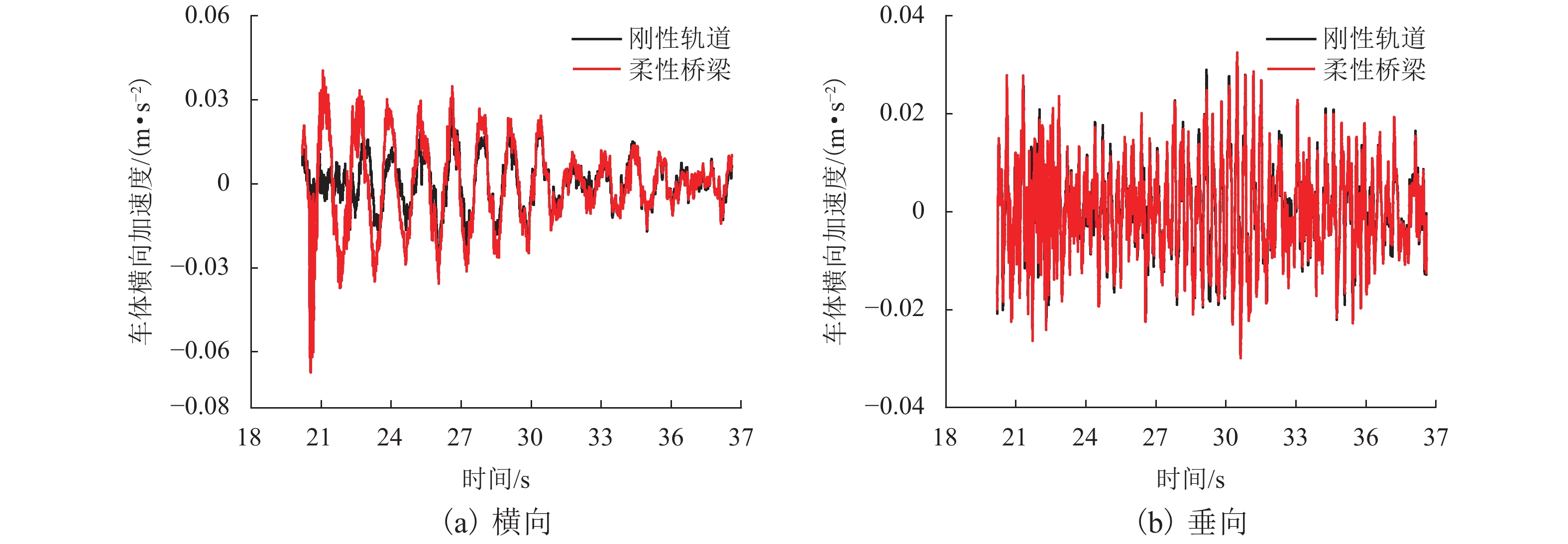
为研究桥梁柔性对中低速磁浮车辆在曲线半径为70.0 m的平曲线上运行时的动态响应影响，对通过柔性桥梁和刚性轨道时的车辆动态响应开展了对比分析. 首先，建立了122个自由度的车辆空间动力学模型，模型中考虑了具有主动悬浮与被动导向特性的二维磁轨关系；其次，利用三维铁木辛柯梁参数化建模方法，建立了由柔性桥梁组成的平曲线有限元模型；最后，通过悬浮力的联系形成了车辆-曲线桥梁系统刚柔耦合动力学模型. 研究结果表明：17.0 m跨径的圆曲线桥梁的自振特性和动位移响应满足相关标准要求；与车辆通过刚性轨道相比，柔性桥梁作用下的车辆系统动态响应更为剧烈，这种差异在车辆系统的横向动态响应上体现明显，而悬浮间隙和车体垂向加速度的响应差异较小，考虑刚性轨道时将高估车辆的曲线通过能力；柔性桥梁和刚性轨道两种模型计算得到的电磁铁最大横向位移不超过6.0 mm，悬浮间隙可在额定值的 ± 4.0 mm内波动，表明在开展对比计算的工况下车辆具有良好的曲线通过性能.
- 中低速磁浮车辆 /
- 曲线通过 /
- 桥梁 /
- 悬浮控制 /
- 动态响应
Abstract:
In order to study the effect of bridge flexibility on the dynamic response of medium-low-speed maglev vehicles running on a horizontal curve with a curve radius of 70.0 m, a comparative analysis of the vehicle dynamic response through a flexible bridge and a rigid track is carried out. Firstly, a spatial dynamics vehicle model with 122 degrees of freedom is established, and the two-dimensional magnet/rail relationship with active levitation and passive guidance characteristics is considered in the model. Secondly, a horizontal curve finite element model consisting of flexible bridges is developed by using a parametric modeling method of three-dimensional Timosheko beam. Finally, the rigid-flexible coupled dynamic model of the vehicle-curve bridge system is constructed with the connection of levitation forces. The results show that, the self-oscillation characteristics and dynamic displacement response of the 17.0 m span circular-curve bridge meet the requirements of relevant standards. Compared with the case of a vehicle passing the rigid track, the dynamic response of the vehicle system under the influence of the flexible bridge is more drastic, and evident in the lateral dynamic response of the vehicle system, while the difference in the response of the levitation gap and the vertical acceleration of the car body is smaller, and the curve passing ability of the vehicle will be overestimated in the case of the rigid track. The maximum lateral displacement of the electromagnet calculated with the flexible bridge and rigid track models does not exceed 6.0 mm, and the levitation gap fluctuates within ±4.0 mm of the rated value, indicating that the vehicle has a good curve passing performance in the comparison analysis.
- medium-low-speed maglev vehicles /
- curve passing /
- bridge /
- levitation control /
- dynamic response

HTML全文

图 1 （悬挂）中置式悬浮架

Figure 1. Levitation frame with mid-set air spring (suspension)

下载: 全尺寸图片幻灯片

图 2 车辆拓扑关系

Figure 2. Topological relationships of vehicle

下载: 全尺寸图片幻灯片

图 3 控制逻辑框图

Figure 3. Block diagram of control logic

下载: 全尺寸图片幻灯片

图 4 柔性桥梁组成及圆曲线桥梁模态

Figure 4. Composition of flexible bridge and modal of circle curved bridge

下载: 全尺寸图片幻灯片

图 5 可视化的车辆-曲线桥梁耦合系统动力学模型

Figure 5. Visualized dynamic model of vehicle-curve bridge coupled system

下载: 全尺寸图片幻灯片

图 6 轨道不平顺

Figure 6. Track irregularity

下载: 全尺寸图片幻灯片

图 7 圆曲线桥梁跨中的动态响应

Figure 7. Dynamic response of mid-span at circular-curve bridge

下载: 全尺寸图片幻灯片

图 8 电磁铁动态响应

Figure 8. Dynamic response of electromagnet

下载: 全尺寸图片幻灯片

图 9 移动滑台和空气弹簧的横向位移

Figure 9. Lateral displacement of sliding table and air spring

下载: 全尺寸图片幻灯片

图 10 车体振动加速度

Figure 10. Vibration acceleration of car body

下载: 全尺寸图片幻灯片

表 1 车辆系统自由度

Table 1. Degrees of freedom of vehicle system

部件	纵向	横向	垂向	侧滚	点头	摇头	备注
悬浮模块	x_lj	y_lj	z_lj	α_lj	β_lj	γ_lj	j = 1~10
防侧滚梁						γ_aj	j = 1~10
吊杆				α_hj	β_hj		j = 1~10
车体	x_c	y_c	z_c	α_c	β_c	γ_c
移动滑台		y_sj					j = 1~6
牵引杆					β_tj	γ_tj	j = 1~10

下载: 导出CSV

表 2 主要计算参数

Table 2. Main calculation parameters

名称	数值
车体质量/kg	17 010
车体绕 x/y/z 轴的转动惯量/（kg•m²）	19 972/349411/351664
悬浮模块质量/kg	1225
悬浮模块绕 x/y/z 轴的转动惯量/（kg•m²）	72/914/908
防侧滚梁质量/kg	27.5
防侧滚梁绕 x/y/z 轴的转动惯量/（kg•m²）	2.28/0.12/2.23
悬浮模块左右中心距/mm	1900
空气弹簧水平刚度/（N•m⁻¹）	1.45 × 10⁵
空气弹簧垂向刚度/（N•m⁻¹）	1.6 × 10⁵

下载: 导出CSV

参考文献(29)

[1]	翟婉明,赵春发. 现代轨道交通工程科技前沿与挑战[J]. 西南交通大学学报,2016,51(2): 209-226. doi: 10.3969/j.issn.0258-2724.2016.02.001 ZHAI Wanming, ZHAO Chunfa. Frontiers and challenges of sciences and technologies in modern railway engineering[J]. Journal of Southwest Jiaotong University, 2016, 51(2): 209-226. doi: 10.3969/j.issn.0258-2724.2016.02.001
[2]	马卫华,罗世辉,张敏,等. 中低速磁浮车辆研究综述[J]. 交通运输工程学报,2021,21(1): 199-216. MA Weihua, LUO Shihui, ZHANG Min, et al. Research review on medium and low speed maglev vehicle[J]. Journal of Traffic and Transportation Engineering, 2021, 21(1): 199-216.
[3]	翟婉明,赵春发. 磁浮车辆/轨道系统动力学(Ⅰ): 磁/轨相互作用及稳定性[J]. 机械工程学报,2005,41(7): 1-10. doi: 10.3321/j.issn:0577-6686.2005.07.001 ZHAI Wanming, ZHAO Chunfa. Dynamics of maglev vehicle/guideway systems (Ⅰ): magnet/rail interaction and system stability[J]. Chinese Journal of Mechanical Engineering, 2005, 41(7): 1-10. doi: 10.3321/j.issn:0577-6686.2005.07.001
[4]	DING J F, YANG X, LONG Z Q. Structure and control design of levitation electromagnet for electromagnetic suspension medium-speed maglev train[J]. Journal of Vibration and Control, 2019, 25(6): 1179-1193. doi: 10.1177/1077546318813405
[5]	龙志强,洪华杰,周晓兵. 磁浮列车的非线性控制问题研究[J]. 控制理论与应用,2003,20(3): 399-402. doi: 10.3969/j.issn.1000-8152.2003.03.016 LONG Zhiqiang, HONG Huajie, ZHOU Xiaobing. Research of nonlinear control for maglev train[J]. Control Theory & Applications, 2003, 20(3): 399-402. doi: 10.3969/j.issn.1000-8152.2003.03.016
[6]	周丹峰,李杰,余佩倡,等. 磁浮交通轨排耦合自激振动分析及自适应控制方法[J]. 自动化学报,2019,45(12): 2328-2343. ZHOU Danfeng, LI Jie, YU Peichang, et al. Analysis and adaptive control of the track induced self-excited vibration for the maglev transport[J]. Acta Automatica Sinica, 2019, 45(12): 2328-2343.
[7]	KONG E, SONG J S, KANG B B, et al. Dynamic response and robust control of coupled maglev vehicle and guideway system[J]. Journal of Sound and Vibration, 2011, 330(25): 6237-6253. doi: 10.1016/j.jsv.2011.05.031
[8]	SUN Y G, XU J Q, CHEN C, et al. Fuzzy H_∞ robust control for magnetic levitation system of maglev vehicles based on T-S fuzzy model: design and experiments[J]. Journal of Intelligent & Fuzzy Systems, 2019, 36(2): 911-922.
[9]	WANG H, ZHONG X B, SHEN G. Analysis and experimental study on the MAGLEV vehicle-guideway interaction based on the full-state feedback theory[J]. Journal of Vibration and Control, 2015, 21(2): 408-416. doi: 10.1177/1077546313488431
[10]	CHEN X H, MA W H, LUO S H. Study on stability and bifurcation of electromagnet-track beam coupling system for EMS maglev vehicle[J]. Nonlinear Dynamics, 2020, 101(4): 2181-2193. doi: 10.1007/s11071-020-05917-8
[11]	ZHAI W M, HAN Z L, CHEN Z W, et al. Train-track-bridge dynamic interaction: a state-of-the-art review[J]. Vehicle System Dynamics, 2019, 57(7): 984-1027. doi: 10.1080/00423114.2019.1605085
[12]	KIM K J, HAN J B, HAN H S, et al. Coupled vibration analysis of maglev vehicle-guideway while standing still or moving at low speeds[J]. Vehicle System Dynamics, 2015, 53(4): 587-601. doi: 10.1080/00423114.2015.1013039
[13]	HAN J B, HAN H S, KIM S S, et al. Design and validation of a slender guideway for maglev vehicle by simulation and experiment[J]. Vehicle System Dynamics, 2016, 54(3): 370-385. doi: 10.1080/00423114.2015.1137957
[14]	LEE J S, KWON S D, KIM M Y, et al. A parametric study on the dynamics of urban transit maglev vehicle running on flexible guideway bridges[J]. Journal of Sound and Vibration, 2009, 328(3): 301-317. doi: 10.1016/j.jsv.2009.08.010
[15]	WANG D X, LI X Z, LIANG L, et al. Influence of the track structure on the vertical dynamic interaction analysis of the low-to-medium-speed maglev train-bridge system[J]. Advances in Structural Engineering, 2019, 22(14): 2937-2950. doi: 10.1177/1369433219854550
[16]	王党雄,李小珍,梁林. 中低速磁浮列车-桥梁系统竖向耦合振动理论分析与试验验证[J]. 土木工程学报,2019,52(8): 81-90. WANG Dangxiong, LI Xiaozhen, LIANG Lin. Theoretical analysis and experimental verification of the vertical coupling vibration of low-to-medium speed maglev train-bridge system[J]. China Civil Engineering Journal, 2019, 52(8): 81-90.
[17]	WANG D X, LI X Z, WANG Y W, et al. Dynamic interaction of the low-to-medium speed maglev train and bridges with different deflection ratios: experimental and numerical analyses[J]. Advances in Structural Engineering, 2020, 23(11): 2399-2413. doi: 10.1177/1369433220913367
[18]	YIM B H, HAN H S, LEE J K, et al. Curving performance simulation of an EMS-type maglev vehicle[J]. Vehicle System Dynamics, 2009, 47(10): 1287-1304. doi: 10.1080/00423110802632071
[19]	赵春发,翟婉明,王其昌. 低速磁浮车辆曲线通过动态响应仿真分析[J]. 中国铁道科学,2005,26(3): 94-98. doi: 10.3321/j.issn:1001-4632.2005.03.020 ZHAO Chunfa, ZHAI Wanming, WANG Qichang. Simulation analysis of the dynamic response of low-speed maglev vehicle curve negotiation[J]. China Railway Science, 2005, 26(3): 94-98. doi: 10.3321/j.issn:1001-4632.2005.03.020
[20]	赵春发,翟婉明. 低速磁浮车辆导向方式及其横向动态特性[J]. 中国铁道科学,2005,26(6): 28-32. doi: 10.3321/j.issn:1001-4632.2005.06.006 ZHAO Chunfa, ZHAI Wanming. Guidance mode and dynamic lateral characteristics of low-speed maglev vehicle[J]. China Railway Science, 2005, 26(6): 28-32. doi: 10.3321/j.issn:1001-4632.2005.06.006
[21]	CUI P, LI J, LIU D S. Carrying capacity for the electromagnetic suspension low-speed maglev train on the horizontal curve[J]. Science China Technological Sciences, 2010, 53(4): 1082-1087. doi: 10.1007/s11431-009-0394-8
[22]	曾佑文,王少华. 三转向架磁悬浮车几何曲线通过分析[J]. 西南交通大学学报,2003,38(3): 282-285. doi: 10.3969/j.issn.0258-2724.2003.03.009 ZENG Youwen, WANG Shaohua. Research on geometrical curve negotiating of three-truck maglev vehicle[J]. Journal of Southwest Jiaotong University, 2003, 38(3): 282-285. doi: 10.3969/j.issn.0258-2724.2003.03.009
[23]	POGORELO D. Differential-algebraic equations in multibody system modeling[J]. Numerical Algorithms, 1998, 19(1): 183-194.
[24]	CHEN X H, MA W H, LUO S H, et al. A vehicle–track beam matching index in EMS maglev transportation system[J]. Archive of Applied Mechanics, 2020, 90(4): 773-787. doi: 10.1007/s00419-019-01638-6
[25]	BRZEZINA W, LANGERHOLC J. Lift and side forces on rectangular pole pieces in two dimensions[J]. Journal of Applied Physics, 1974, 45(4): 1869-1872. doi: 10.1063/1.1663505
[26]	德米特里 · 波戈列洛夫,雷强,根纳季 · 米克希夫,等. 基于UM的磁浮列车-轨道梁耦合振动仿真程序开发[J]. 计算机辅助工程,2019,28(1): 28-35. POGORELOV Dmitry, LEI Qiang, MIKHEEV Gennady, et al. Development of dynamics simulation program for coupling vibration of maglev train-track beam based on UM[J]. Computer Aided Engineering, 2019, 28(1): 28-35.
[27]	中国铁路设计集团有限公司, 中铁第四勘察设计院集团有限公司, 中车同月研究院有限公司. 磁浮铁路技术标准（试行）: TB 10630—2019[S]. 北京: 中国铁道出版社有限公司, 2019.
[28]	PARK K C. An improved stiffly stable method for direct integration of nonlinear structural dynamic equations[J]. Journal of Applied Mechanics, 1975, 42(2): 464-470. doi: 10.1115/1.3423600
[29]	中华人民共和国住房和城乡建设部. 中低速磁浮交通设计规范: CJJ/T 262—2017[S]. 北京: 中国建筑工业出版社, 2017.