Stability Control of Electrodynamic Suspension with Permanent Magnet and Electromagnet Hybrid Halbach Array
-
摘要:
为了研究不同控制方法下永磁电磁混合Halbach阵列的电动悬浮稳定性，首先，利用电磁场理论对系统悬浮力2D解析式进行了推导，并搭建有限元模型对其进行了验证；其次，建立了系统垂向动力学模型，设计了基于气隙反馈的定气隙PID控制器和变气隙PID控制器；最后，仿真分析了系统受到外界扰动时的悬浮气隙及线圈电流波形. 研究结果表明：当系统受到1 mm轨道沉降扰动时，两种控制器均能使系统稳定运行于额定状态，且动态过程一致；当系统受到 ±1000 N扰动力作用时，定气隙PID控制器可使系统稳定悬浮于额定气隙30 mm位置，且稳态线圈电流分别为2.12 A/mm2和 −2.17 A/mm2，变气隙PID控制器则使系统分别稳定悬浮于28.5 mm及31.6 mm位置，且稳态线圈电流均为0.
Abstract:In order to study the suspension stability of the electrodynamic suspension (EDS) with permanent magnet (PM) and electromagnet hybrid halbach array under different control methods, Firstly, the 2D levitation force analytical expression was derived by using the electromagnetic field theory and the corresponding finite element model (FEM) was built to verify the suspension force analytical expression. Secondly, the vertical dynamic model of the system was established, and the fixed air gap PID controller and variable air gap PID controller based on the air gap feedback were designed respectively. Finally, the system suspension air gap and current density waveforms under external disturbances were simulated and analyzed. The simulation results indicate that both controllers can make the system suspend stably at the rated state and have the same dynamic process under the 1 mm track settlement disturbance. When the system is disturbed by the ± 1000 N disturbing force, the fixed air gap PID controller can make the system suspend stably at the 30 mm rated air gap, and apply the steady state coil current of 2.12 A/mm2 and −2.17 A/mm2 respectively. The variable air gap PID controller makes the system suspend stably at 28.5 mm and 31.6 mm respectively, and applies the steady-state coil current of 0.
-
Skyline查询是解决计算机多目标优化问题的一类重要方法，由Borzony等[1]提出，目前在位置导航、数据挖掘和推荐算法中具有越来越广泛的应用[2-5]. 尽管skyline查询机制从理论上讲能够保证隐私，但在实践中，攻击者通过重复攻击仍然可以获得个人信息[6]. 因此，需要进一步研究该机制中防止重复攻击的问题. skyline查询的结果为没有被其他任何点支配的对象，也正是因为这一特性，用户的隐私容易泄露[7]. 为进行数据的隐私保护，Dwork[8]提出了一种基于差分隐私保护的方法，通过对数据进行一般的统计分析，为隐私保护提供定量的评估技术，从而实现差分隐私保护的功能. 文献[9]提出了一种操作方便、定量准确的方法，可以在执行独立的差异私有机制的过程中跟踪累积的隐私损失. 文献[10]提出了一种基于环境中整体迹线和已发布迹线之间相互联系的方法，该方法可以在给定查询约束的情况下将迹线隐私泄漏的几率最小化. 文献[11]提出了一种设置线性查询数量上限的方法，该方法能够找到最优的线性查询. 基于差分隐私的查询机制有两种，分别是拉普拉斯机制[12]和指数机制[13]，基于差分隐私的数据挖掘方法广泛应用于频繁模式挖掘[14]、MapReduce大数据分析查询[15]、智能权重截取算法[16]和智能电网[17].
为了解决差分隐私保护机制中重复攻击会泄露用户隐私的问题，国内外研究人员提出了许多解决方法. 文献[18]为解决二维多媒体数据不均匀和精度降低的问题，提出了一种基于标准偏差圆半径的差分隐私噪声动态分配算法. 文献[19]使用传统搜索未涵盖的属性关联来调查新的隐私威胁，通过配置差异隐私的噪声参数来减轻周期滑动推理攻击对差分隐私的影响. 文献[20]通过对样本数据进行训练，获得一个对抗性网络数据模型，将噪声数据添加到真实数据集中，可以在高斯分布下实现不同等级的隐私保护. 文献[21]提出了一种用于位置数据隐私的隐私保护方法，该方法可满足不同的隐私约束. 但是，目前这些方法都无法满足skyline查询中隐私保护的要求，并且无法动态设定隐私预算值以实现不同等级的隐私保护级别. 文献[22]提出了一种设定查询上限的高效方法，通过计算准确性的期望值与可用于补偿个人的预算值之间的平均值来确定计算查询上限. 文献[23]提出了一种基于环境中整体迹线和已发布迹线之间的相互联系的方法，通过计算整体位置迹线的隐私度量值来确定接下来最优的位置迹线方案，该方法可以在给定查询约束的情况下将迹线隐私泄漏几率最小化. 文献[24]提出了一种设置线性查询数量上限的方法，通过找到最优的线性查询，设置查询数量的上限，使得最优的线性查询也无法泄露过多的隐私. 但是仍然无法在分级查询中动态改变隐私预算值的同时对查询次数的上限进行调整. 因此，文献[25]提出了一种有效且可保护隐私的在线医疗基础诊断框架，在该框架内通过skyline查询，用户可以准确访问在线医疗诊断服务，而无需泄露他们的医疗数据.
综上所述，针对传统skyline查询方法无法有效地解决用户隐私泄露的问题，提出了一种基于差分隐私保护的skyline查询方法. 本文的主要贡献包括3个方面：
1） 为提高skyline查询的效率，提出最优主导页的概念. 最优主导页能够针对skyline查询结果的分页特性，确定查询范围的数据隐私保护等级. 针对skyline查询的特性提出页敏感度的概念，页敏感度能够满足ε-差分隐私.
2） 为解决因噪音导致有效查询结果的范围无法量化的问题，引进置信区间和置信率的概念；为解决不同信任等级的用户查询结果相同会泄露数据隐私的问题，提出一种基于置信率的隐私预算值调节方法，对不同的信任等级的用户设定不同的隐私预算值，实现数据的分级保护.
3） 为解决攻击次数过多导致用户隐私泄露的问题，提出调整隐私预算和置信率，限制查询次数的策略，从而保护隐私数据.
1. 基本定义
定义1 全局敏感度[12]. 设有函数f ：T→Rd ，T为输入数据集，输出为d维实数向量. 对于T的任意邻近数据集Ta ，函数f的全局敏感度GSf = max{T, Ta} || f(T) −f(Ta) ||1 ，其中，|| f(T) −f(Ta) || 1 是 f(T) 和 f(Ta) 之间的1-阶范数距离，表示相差的个数，这里个数只能为1，若 || f(T)−f(Ta) ||1 =1，表示相差一个.
函数的全局敏感度由函数本身决定，不同的函数会有不同的全局敏感度. 一些函数具有较小的全局敏感度，因此，只需加入少量噪声即可掩盖因一个记录被删除对查询结果所产生的影响，从而实现差分隐私保护. 但是仍然无法满足某些需求，例如求平均值和中位数等函数，则具有较大的全局敏感度. 此时，敏感度可能是一个很大的值，无法满足隐私保护的要求. 为解决该问题，进一步提出了页敏感度的概念.
定义2 页敏感度. 设有函数 f ：T→Rd ，T为一个输入数据集，输出为d维实数向量，对于输入数据集T和任意的邻近数据集Ta， LSf (T) = max{Ta}× || f(T) −f(Ta) ||1 ，称为函数f在数据集T上的页敏感度.
为进一步调节页敏感度，合理设置隐私预算的值，引入了基于差异化拉普拉斯分布的置信区间和置信率的概念. 置信区间和置信率与隐私预算有关，隐私预算的大小决定隐私保护的效果，如果所添加的噪声是有效的，则一次查询无法获得用户的隐私. 但是，如果攻击者进行多次重复攻击，并且噪声分布符合拉普拉斯分布，实际结果就会在某一个区间. 为进一步量化该区间范围，给出置信区间的定义如定义3所示.
定义3 置信区间. 符合拉普拉斯分布的噪声在某一个区间范围内，如果用
$ \varphi $ 代表置信区间的一半长度，则$ [- \varphi , \varphi ]$ 为置信区间[24].由拉普拉斯分布知置信区间是对称的，则可以推断出被攻击数据的私有数据，并且可以通过累计函数计算攻击的成功率A(T)，实际结果为
A(T)=f(T)+L(ε/Δf), (1) 式中：ε为隐私预算值；
$ \Delta $ f为全局敏感度；$ \mathcal{L}\left({\text{•}} \right) $ 为拉普拉斯变换.$ \mathcal{L}(\varepsilon /\Delta f) $ 的置信区间为$ [\mu - \varphi ,\;\mu + \varphi ]$ ，其中，$ \mu $ 为位置参数，是相对于参数$ \varphi $ 对称的未知参数. 为量化计算结果在置信区间的概率，进一步给出了置信率的概念，如定义4所示.定义4 置信率. f(T)的查询结果落在置信区间
$ [\mu- \varphi ,\;\mu+\varphi ] $ 的概率为置信率，则置信率通过累计函数计算为[24]F(μ−φ,μ+φ)=1−1/2exp(sgn(−φε/Δf)). (2) 根据置信率进一步量化隐私预算值ε的上界，设p为隐私泄露的概率，则
ε⩽Δfln(2(1−p))sgn(−φ). (3) 隐私预算值上界可以限制隐私预算值的大小，并且与置信率相关. 查询结果有两种可能性，分别是查询结果落在置信区间和查询结果不落在置信区间，两种结果互斥. 设攻击者进行查询的总次数为C，在置信区间内的查询结果次数为m，m为大于0的整数，则攻击成功的概率为
pc=m+1∑i=1Cm+i2m+1(1−12e−ε2)m+i(12e−ε2)m−i+1. (4) 因此，若对攻击者的成功率进行限制，使其不大于某个阈值，则能够确定隐私预算的取值范围. 隐私预算 ε 的选择与查询的页敏感度 LSf (T)、攻击者的总查询次数C、置信区间
$ [-\varphi ,\;\varphi ]$ 和攻击成功率pc有关，隐私预算取值范围的计算如式（5）所示.0<ε⩽m+1∑i=1CiC(1−12exp(sgn(φ)2LSf(T)))i(12exp(sgn(φ)2LSf(T)))C−i. (5) 理想的情况下，成功的事件和不成功的事件互斥，ε的值接近1/2时，则成功率近似为50%. 假设置信区间为
$ [1/2-\omega ,1/2 + \omega ] $ ，其中，$ \omega $ 为用户设定的成功率参数，取决于数据的隐私性和能够承受攻击的上限. 当查询结果在置信区间的成功率大于1/2时，可对页敏感度进行调整，即降低页敏感度，增加隐私保护等级，因此，置信区间成功率可以控制在用户设定的$ [1/2-\omega , 1/2 + \omega ] $ 区间内.最后，为对skyline的查询结果进行优化，给出最优主导页的概念如定义5所示.
定义5 最优主导页. 根据支配页将skyline查询的输入数据集T分为h个子数据集Tsub1, Tsub2, … , Tsubh ，在一次具体查询中，如果查询数据集为部分子数据集Tsubk, Tsubk + 1, … , Tsubk + z ，其中 k 和 z为正整数且0 < k + z ≤ h，则该查询的最优主导页为Tsubk.
2. 基于差分隐私保护的skyline查询方法
skyline查询结果通常是隐私数据，故用户的隐私更容易泄露. 为保护用户隐私，提出了基于差分隐私保护的skyline查询方法，所提方法的主要思想为：根据查询对象的范围确定查询的最优主导页，从而确定页敏感度；根据查询者的查询范围、查询次数评估查询者的信任等级，限制查询次数；根据页敏感度和信任等级进一步确定隐私预算，给出查询结果.
2.1 最优主导页的计算方法
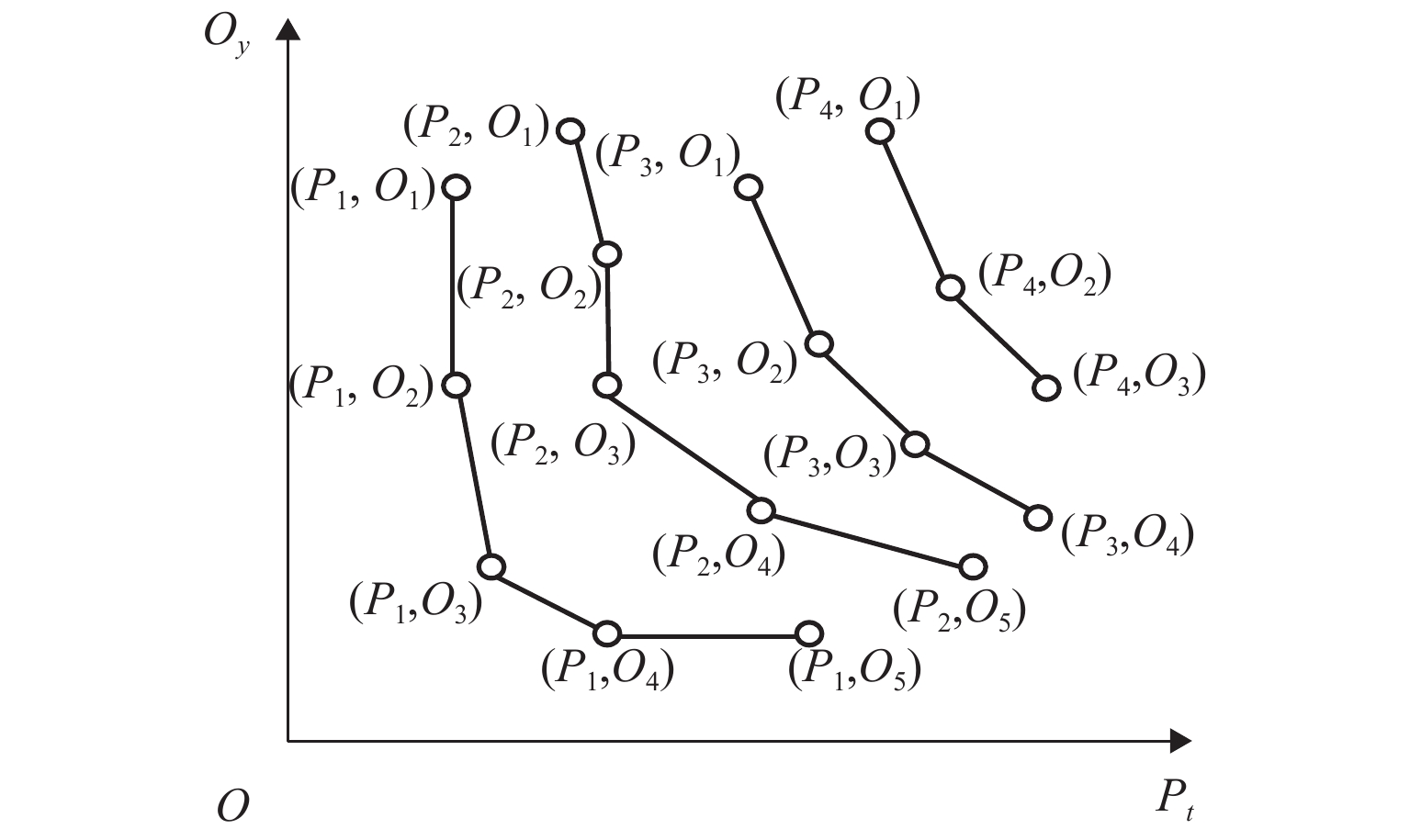
最优主导页是指在一次skyline查询范围中，隐私最容易被泄露的某一页查询结果. 最优主导页的计算过程为：首先，通过skyline查询获得查询结果，将隐私最容易被泄露的某一页查询结果确定为最优主导页；其次，遍历数据集并实时更新查询结果；后续依据查询范围的改变再动态更新最优主导页. 如图1所示，图中，Pt、Oy分别为第t页、第y个对象.
首先，利用skyline分页查询对所有的数据点进行分页，查到每一页所有不被支配的数据点，每一页为一个隐私泄露等级的子数据集.
第一页的第一个对象记为(P1,O1)，第一页的对象子数据集为{(P1,O1), (P1,O2), (P1,O3), (P1,O4), (P1,O5)}，以此类推，每一页查询结果如表1所示.
表 1 skyline分页查询结果Table 1. Skyline paged query results页 结果 1 {(P1, O1), (P1, O2), (P1, O3), (P1, O4), (P1, O5)} 2 {(P2, O1), (P2, O2), (P2,O3), (P2, O4), (P2, O5)} 3 {(P3, O1), (P3, O2), (P3, O3), (P3, O4)} 4 {(P4,O1), (P4, O2), (P4, O3)} 根据所有对象的属性向量计算第i （i = 1, 2, …）页skyline查询结果，第1页为首次查询确定的最优主导页，每一次查询的最优主导页都代表最容易泄露当前数据隐私的子数据集.
2.2 页敏感度的计算方法
在skyline查询过程中，局部敏感度计算的过程比较复杂，全局敏感度无法对子集进行分类保护；查询次数和查询结果的隐私泄露程度具有正相关性，页敏感度能够调节两者相关性的大小.
基于差分隐私保护的查询通过添加噪音干扰查询结果，从而保护用户隐私. 查询机制有两种，分别是拉普拉斯机制和指数机制，其中拉普拉斯机制是一种噪音干扰机制，噪音是一种满足拉普拉斯分布（Laplace distribution）的函数. 基于拉普拉斯机制的skyline查询的页敏感度为 LSf (T)时，满足ε-差分隐私.
基于以上论述，所提计算页敏感度算法 （page sensitivity calculation algorithm，PSC_A）的主要思想为：首先，在预处理阶段通过skyline查询结果初步确定第一次查询的最优主导页，并计算页敏感度；其次，在每次查询时遍历数据集，将当前计算的页数与最优主导页进行比较，更新最优主导页，最终，输出页敏感度的值. PSC_A如算法1所示.
算法1 PSC_A /*页敏感度计算算法*/
输入：数据集T.
输出：页敏感度 LSf (T).
1） 执行skyline查询，通过skyline查询结果初步确定第一次查询的最优主导页；
2） 对skyline查询数据集T进行遍历，计算每一个对象的页数；
3） 将当前计算的页数与最优主导页进行比较；
4） 如果当前数据对象的页数小于最优主导页，则更新最优主导页；
5） 计算当前最优主导页的页敏感度 LSf (T)；
6） 返回页敏感度 LSf (T).
2.3 基于置信率的隐私预算值调节方法
针对不同的隐私泄露等级的子数据集，为了差异性地保护隐私，利用基于拉普拉斯分布的差分隐私保护机制保护数据隐私. 传统的隐私保护方法可以通过引入随机性增加干扰，达到对隐私的有效保护. 设x为查询参数变量，Nnoise是服从某种随机分布的噪声，则f(x) ＝ Ccount(x) + Nnoise，其中，Ccount(x)为针对x的统计函数. 在传统的差分隐私保护方法中，无法对输出的结果进行特定的处理，针对skyline分页查询结果集中的数据隐私保护等级不同的问题，为进一步对不同隐私等级的数据进行分级调节，提出基于置信率的隐私预算值调节方法.
利用拉普拉斯机制执行ε-差分隐私保护，将满足拉普拉斯分布的随机噪声添加到查询结果中，拉普拉斯机制满足
A(T)=f(T)+lap(ε/Δf). (6) 拉普拉斯噪声计算公式为
lap(ε/Δf)=f(x)=1/(2b)exp(−|x−μ|/b), (7) 式中：b > 0 为尺度参数.
设b = ε/
$ \Delta $ f ，$ \mu $ = 0，则f(x)=ε/(2Δf)exp(−|x|Δf/ε). (8) 对于任意绝对值变量，累计函数为
F(x)=1/2+1/2sgn(1−exp(−|x|Δf/ε)). (9) 添加的噪声大小与隐私预算值ε和
$ \Delta $ f密切相关，ε的取值越小，隐私保护效果越好，但是数据的有效性越低；ε的取值越大，数据的隐私保护则效果越差，但是数据的有效性越高. 基于以上论述分析，所提出的基于置信率的隐私预算值调节算法（privacy budget adjustment algorithm for confidence rate，PBAACR_A）如算法2所示.算法2 PBAACR_A /*隐私预算值调节算法*/
输入：查询数据集T, 置信区间
$ [1/2- \omega ,1/2 + \omega ]， $ 查询次数C.输出：隐私预算值.
1） 调用PSC_A算法计算页敏感度；
2） 针对置信区间
$ [1/2- \omega , 1/2 + \omega] $ ，利用页敏感度和式（2）计算置信率；3） 利用式（3）计算隐私预算值；
4） 基于拉普拉斯机制执行ε-差分隐私，利用拉普拉斯噪声计算公式（式（7））将满足拉普拉斯分布的随机噪声添加到查询结果中；
5） 对置信区间
$ [1/2- \omega , 1/2 + \omega] $ 的隐私预算值进行判断，如果满足该置信区间的置信率要求，则输出隐私预算值；否则，隐私预算值设置为0.2.4 基于差分隐私保护的skyline查询算法
基于页敏感度计算和隐私预算值调节方法，针对skyline查询的次数过多导致用户隐私可能泄露的情况，可采取限制用户查询次数的策略加大隐私保护力度. 在置信区间为
$ [1/2- \omega , 1/2 + \omega] $ 的情况下可根据隐私预算值和全局敏感度计算出置信率. 查询结果满足ε-差分隐私的查询次数上限设为c，当查询次数达到c时，需要对用户信任等级和隐私参数重新计算，再利用PBAACR_A算法对隐私预算值进行调节，最终得到满足隐私保护要求的skyline查询结果.进一步给出基于差分隐私保护的skyline查询算法（skyline query algorithm for differential privacy protection，SQADP_A ），该算法首先计算隐私预算值；其次，基于隐私预算值更新查询次数上限；对每次查询结果进行判断；查询完成后再计算页敏感度；最后，利用拉普拉斯机制添加噪音，输出skyline查询结果. SQADP_A 如算法3所示.
算法3 SQADP_A /*基于差分隐私保护的skyline查询算法*/
输入：查询数据集T，置信区间
$ [1/2- \omega , 1/2 + \omega] $ ，查询总次数C，满足ε-差分隐私的查询次数最大值c.输出：skyline查询结果.
1） 调用PBAACR_A算法得出隐私预算值；
2） 判断隐私预算值，若隐私预算值为0，则更新查询次数上限，重新计算隐私预算值；若隐私预算值不为0，则增加查询次数；
3） 基于置信区间
$ [1/2- \omega , 1/2 + \omega] $ 计算置信率；4） 遍历查询数据集T中的所有子数据集；
5） 针对相邻子数据集进行skyline查询，得到查询结果集；
6） 计算页敏感度；
7） 利用拉普拉斯机制，根据隐私预算值和页敏感度对查询结果集添加噪音；
8） 发布skyline查询结果.
3. 实验评估
3.1 实验环境
为更好地保护隐私，本实验加入人工合成的人名、性别和年龄等信息. 人名为随机生成的3个汉字，性别为男或者女，年龄为0 ~ 100周岁的整数，符合正态分布. 实验环境为Microsoft Windows 10，Core (TM) i7- 3537U CPU@ 2.00 GHz （2501 MHz）处理器，4 GB内存. 假设人名、性别和年龄均为隐私数据，本实验分析skyline查询的效率、查询结果的可靠性以及查询结果的隐私泄露程度.
为构造对比算法，对文献[20]所提算法和文献[21]所提算法的关键步骤进行适当的修改. 在文献[20]所提算法进行剪枝之前增加了最优主导页的计算，并将敏感度更改为页敏感度，简称为OGWP （optimizing GAN obfuscator with pruning）算法；在文献[21]所提算法进行信息熵的计算之前增加最优主导页的计算，并将敏感度的计算更改为动态调节敏感度，简称为FOQIL （find the optimal quantization interval length）算法. 本节将本文所提SQADP_A算法与OGWP算法、FOQIL算法进行对比实验，验证所提算法的可行性和有效性.
3.2 实验结果分析
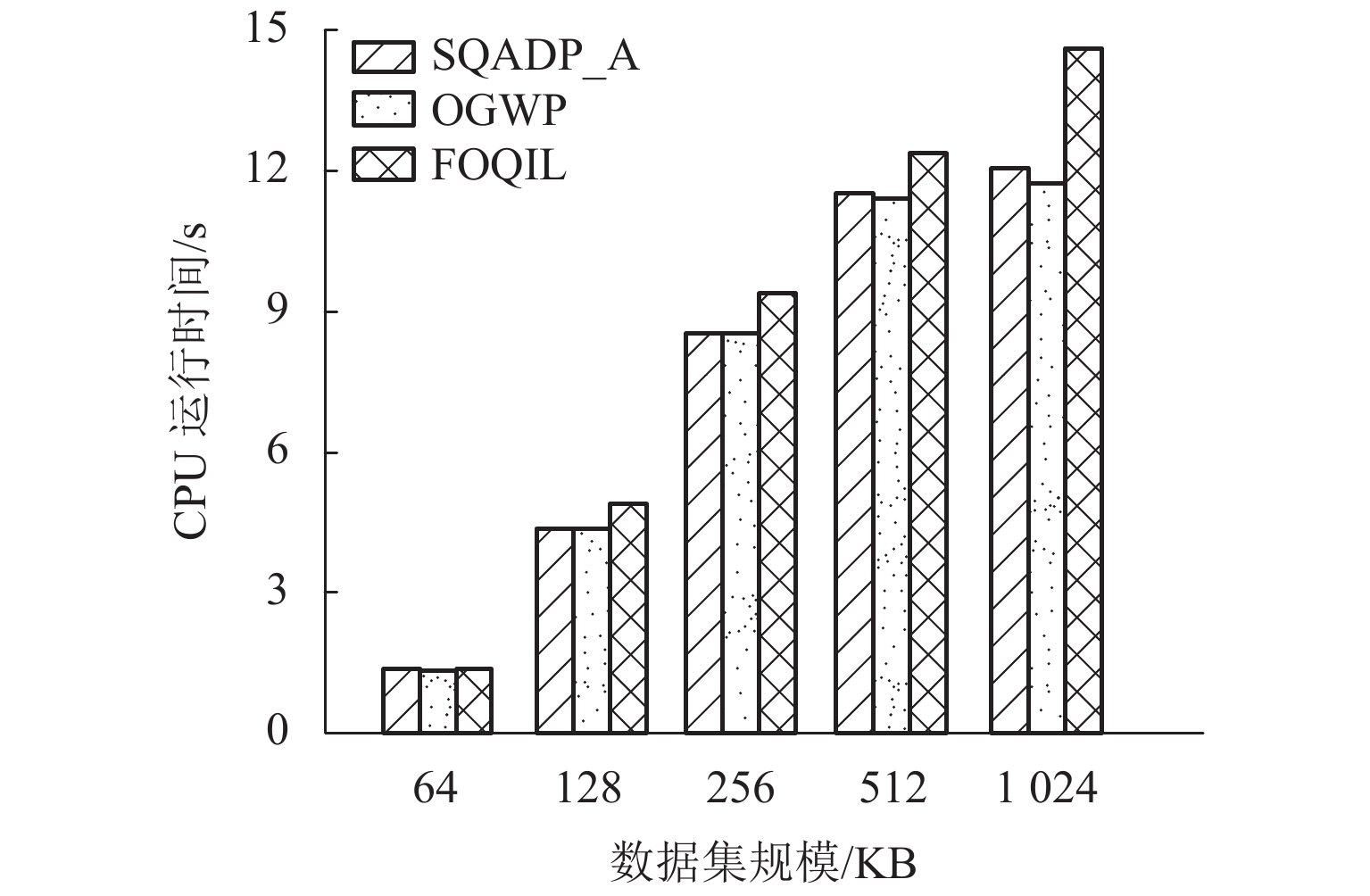
实验1 本实验主要对比3种算法的数据规模对算法执行时间的影响. 图2展示了数据集对象数量从64 kB增长到1024 kB时3种算法的查询效率对比结果，分别分析数据规模的不同对CPU运行时间的影响. 由于剪枝算法能够剪掉一部分无效对象，且最优主导页是动态更新的，所以3种算法在数据规模较小的时候相差不大，但是随着数据规模的增加，FOQIL算法需要额外处理的数据量较多，因此，对很多无效的对象进行最优主导页和信息熵的计算量也增加，查询效率相对较低. 梯度修剪策略由于没有涉及到大量的复杂计算，而是基于高斯分布针对不同场景进行差异化剪枝，因此，OGWP算法效率较高.
实验2 本实验主要评估数据规模对查询结果可靠性的影响. SQADP_A算法依据剪枝规则和存在概率排序规则进行查询. 实验中数据项泄露数为结果集中隐私数据数量，因变量为数据集的规模，在5个不同规模的数据集上进行查询. 如图3所示，因为每次skyline查询的结果输出后，才作为计算页敏感度的剪枝结果，所以SQADP_A算法的输出结果集的无效数据最少. 与SQADP_A算法相比，FOQIL算法的查询结果隐私项较少，隐私保护等级较高，但是由于涉及到大量的计算，查询效率较低. OGWP算法输出结果的隐私数据数量高于FOQIL算法和SQADP_A算法，隐私保护效果最差. 因此，由实验结果可知：如果不考虑查询时间的影响，FOQIL算法和SQADP_A算法更能保护用户隐私. 此外，虽然FOQIL算法和SQADP_A算法的隐私保护等级相差不大，但是，SQADP_A算法的查询速度更快. 因此，随着数据规模的增加，SQADP_A算法能在查询速度较快的情况下增强查询结果的隐私保护.
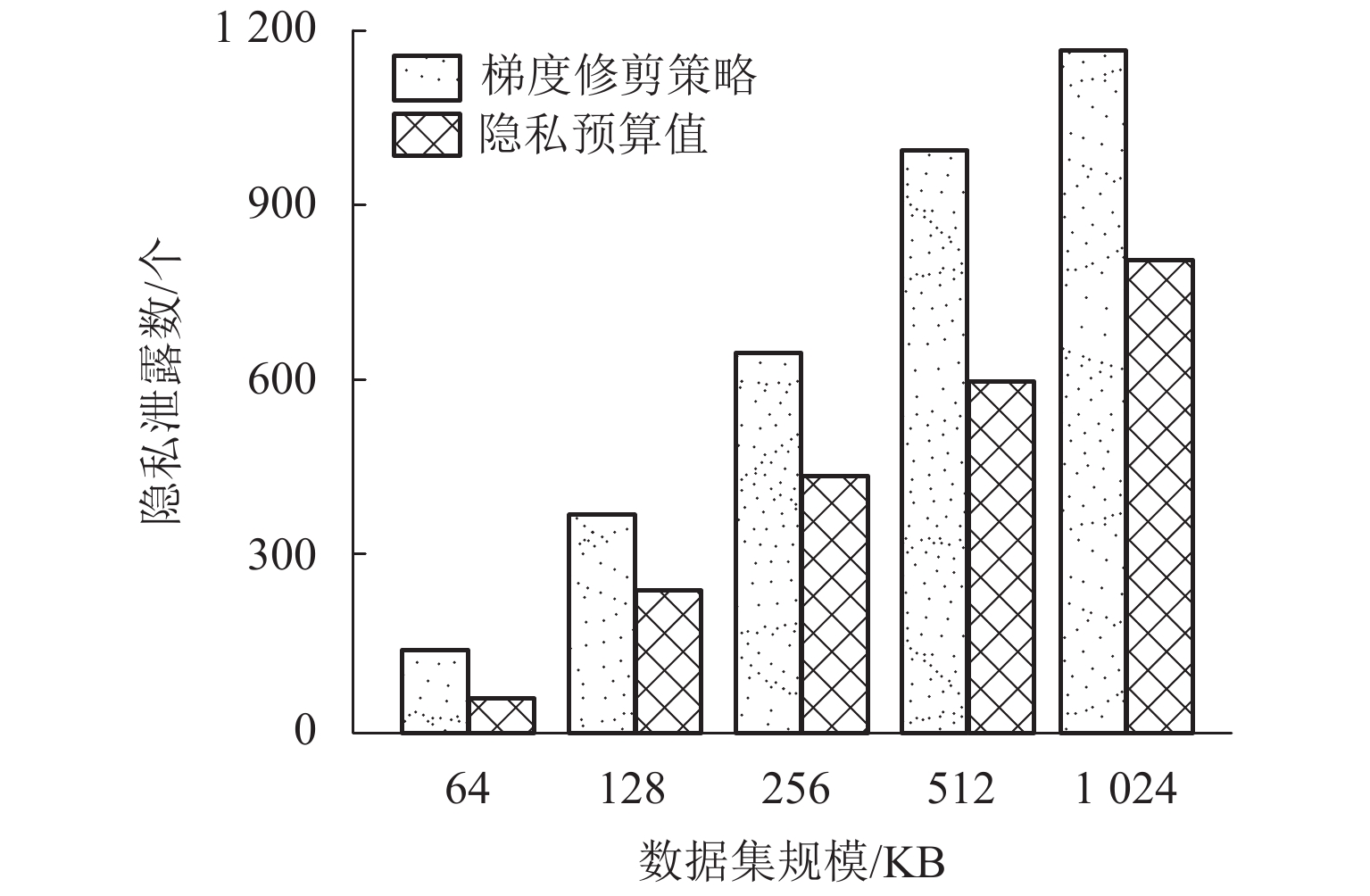
实验3 本实验评估了隐私预算值调节策略和梯度修剪策略对算法输出结果集的隐私泄露程度的影响. 实验中采用控制变量法保证隐私预算值计算的策略不同，结果如 图4所示. 由图4可知：两种查询算法在skyline查询过程中采用不同的策略，其查询结果集中的有效的隐私泄露数具有显著差异；隐私预算值调节策略更适应于对skyline查询隐私保护要求较高的场所，skyline查询的查询结果为没有被其他任何点支配的对象，关于skyline查询的查询结果通常是隐私数据，因此，这种方式可以用较小的时间代价换取隐私保护等级的增加；而梯度修剪策略虽然具有较快的查询速度，但是隐私泄露程度随着数据规模的增加而快速增加. 故如果对skyline查询结果的隐私保护有较高要求则可以采用隐私预算值调节策略，对查询速度有较高要求则可以采用梯度修剪策略.
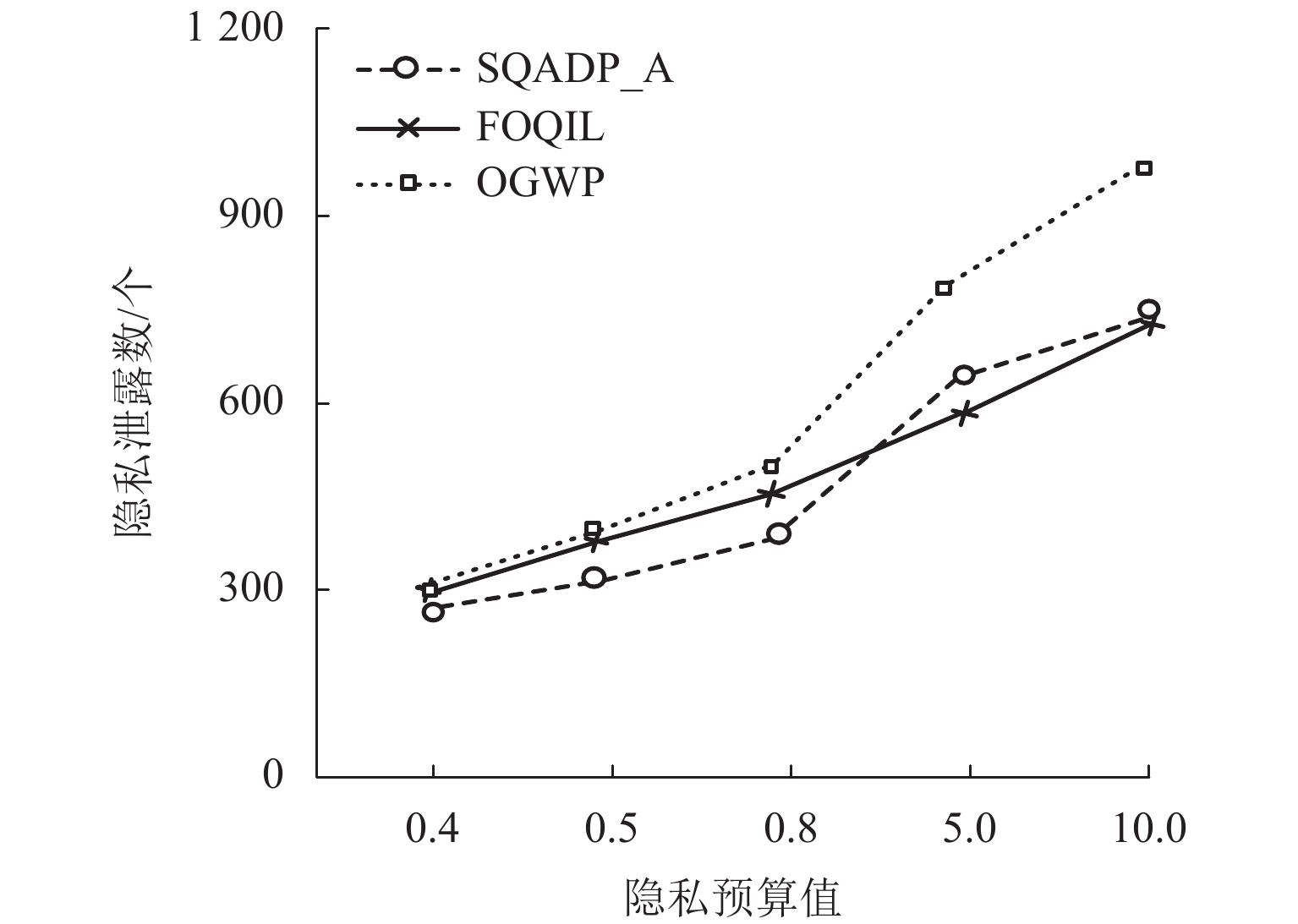
图 4 两种策略对查询结果隐私泄露程度的影响Figure 4. Effect of two strategies on the degree of privacy disclosure of query results实验4 本实验评估了3种算法中的隐私预算值设定对skyline查询结果集中隐私泄露程度的影响，数据规模为256 kB，隐私预算值分别设为10.0、5.0、0.8、0.5、0.4，实验结果如图5所示. 由实验结果可知：隐私预算值设定较低的情况下3种算法的隐私泄露程度没有明显的区别，3种算法在隐私预算设定为0.4时的隐私泄露的差距较小，但是随着隐私预算值设定值的增加，SQADP_A算法的隐私泄露程度逐渐收敛，并且在隐私预算值较小（小于5.0）的情况下，SQADP_A算法的隐私保护效果最好. 隐私预算设定值为10.0时，OGWP算法的隐私泄露程度较高，随着隐私预算值的增加，FOQIL算法的隐私保护效果有降低的趋势.
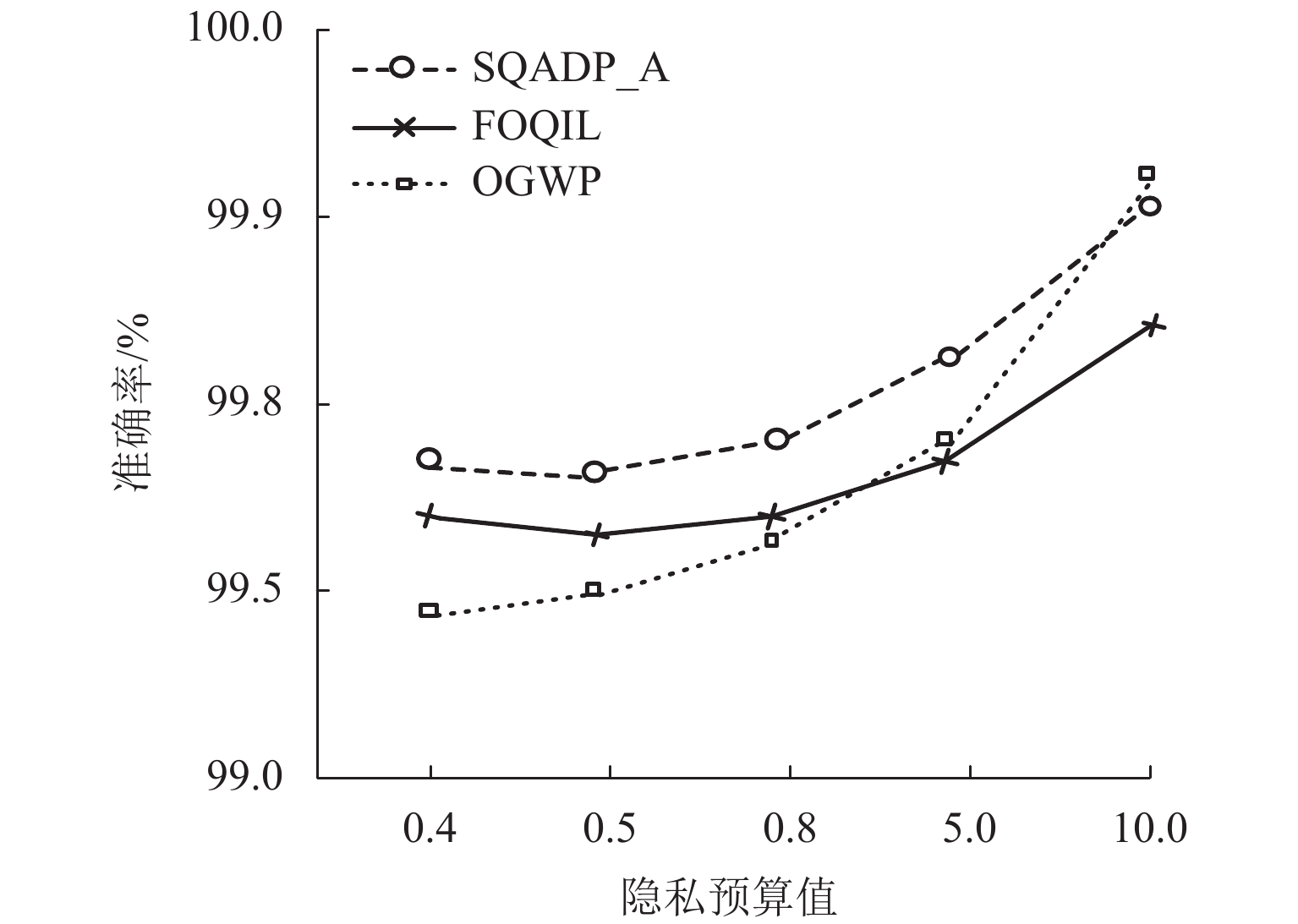
实验5 本实验评估了3种算法中的隐私预算值设定对skyline查询结果集中准确率的影响，实验结果如图6所示. 由图6可知：隐私预算值设定较低的情况下，OGWP算法的查询准确率最低；随着隐私预算值的增加，OGWP算法的隐私保护效果变差，OGWP算法的查询准确率却有所提高. 在隐私预算值设定较低的情况下，SQADP_A算法的查询准确率最高，当隐私预算设定为0.8以内时其查询准确率没有明显的变化，当隐私预算值设定大于0.8时，查询准确率有明显变高的趋势. FOQIL算法在隐私预算设定较低的时候也有较高的准确率，隐私预算值设定为0.8以内时，准确率变化依旧不明显，甚至有降低的趋势；当隐私预算的值大于0.8后准确率逐渐增加. 由实验可知：在skyline查询结果中，SQADP_A算法的准确率相对最高.
图 6 隐私预算值对skyline查询结果集中准确率的影响Figure 6. Effect of privacy budget value on the accuracy of skyline query result set4. 结 论
skyline查询的隐私保护问题受到越来越广泛的关注，差分隐私能够有效地保护skyline查询结果中不同子结果页的隐私. 本文研究了基于差分隐私保护的skyline查询方法，提出了有效的页敏感度计算方法和隐私预算值调节策略. 页敏感度的计算能够有效地适用于skyline查询方法，基于置信率和置信区间的隐私预算值调节策略确保了数据对象的有效性. 最后给出了基于拉普拉斯机制进行查询结果隐私保护的SQADP_A算法. 通过实验表明：所提方法能减少skyline查询结果的隐私泄露数量，为用户提供有效的隐私保护. 未来将深入研究不确定高维skyline查询过程中的数据隐私问题，使得在执行多次查询时，查询结果不仅能保证数据的有效性，也能保证数据的隐私性.
-
图 1 永磁电磁混合Halbach阵列电动悬浮示意
Figure 1. Diagram of PM and electromagnet hybrid Halbach array EDS system
图 7 悬浮气隙及线圈电流密度(变气隙)
Figure 7. Suspension air gap and coil current density (variable air gap )
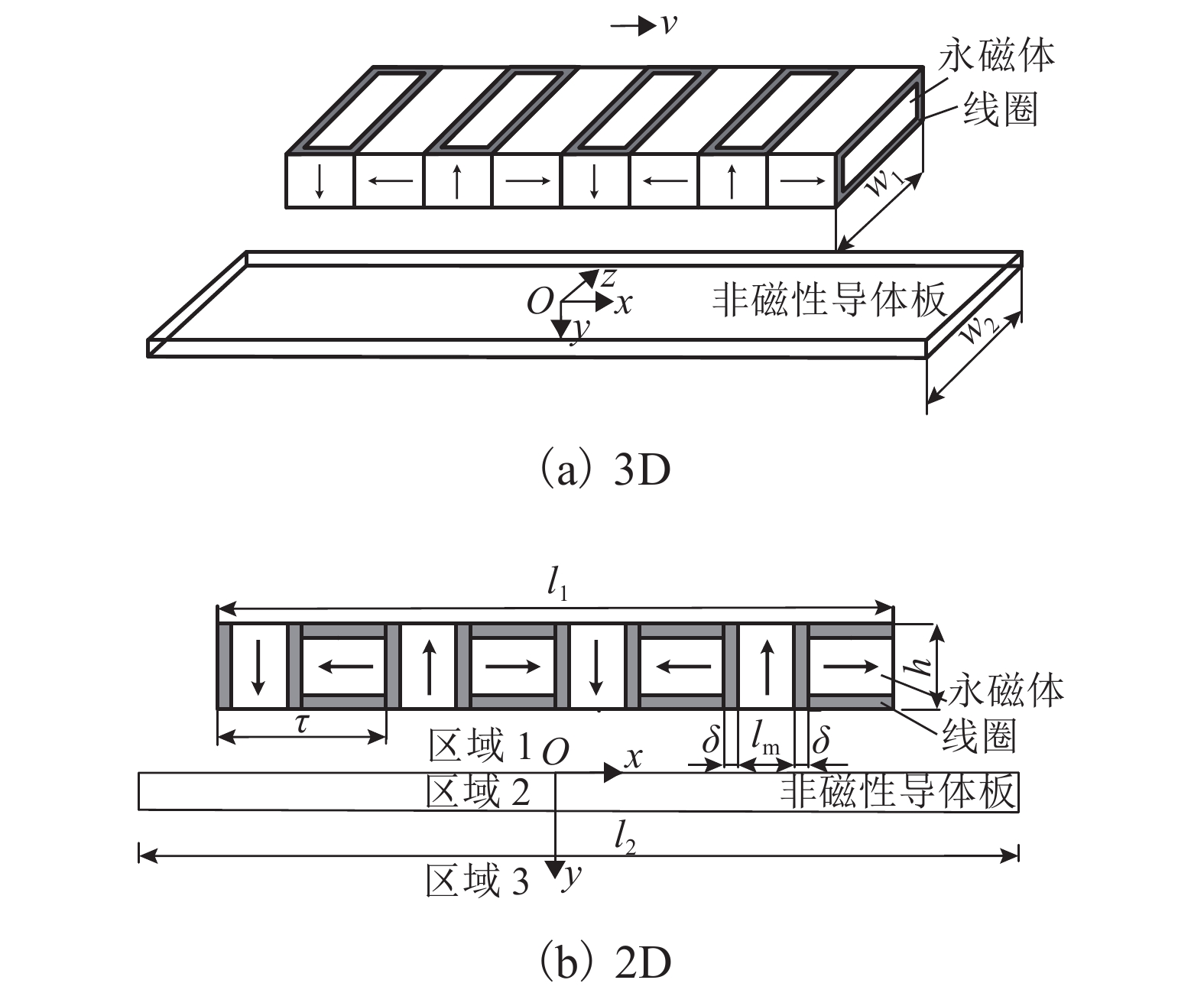
表 1 模型参数
Table 1. Model parameters
参数 数值 参数 数值 永磁体剩磁 Br/T 1.25 线圈宽度/mm 10 永磁体横向宽度/mm 200 线圈电流体密度 J/
（ A•mm−2)）6 永磁体长度/mm 80 导体板横向宽度/mm 200 永磁体高度/mm 100 导体板长度/mm 1600 一对极模块数 m 4 导体板厚度 d/mm 20 阵列总长度/mm 800 导体板电导率/
（S•m−1）3.8 × 107 阵列模块数 N 8 气隙 g/mm 30 
下载: 导出CSV
-
[1] POST R F, RYUTOV D. The Inductrack concept: A new approach to magnetic levitation[R]. Springfield: Office of Scientific and Technical Information (OSTI), 1996. [2] 罗成,张昆仑,靖永志. 新型Halbach阵列永磁电动悬浮系统垂向稳定性[J]. 交通运输工程学报,2019,19(2): 101-109. doi: 10.3969/j.issn.1671-1637.2019.02.010LUO Cheng, ZHANG Kunlun, JING Yongzhi. Vertical stability of permanent magnet EDS system with novel Halbach array[J]. Journal of Traffic and Transportation Engineering, 2019, 19(2): 101-109. doi: 10.3969/j.issn.1671-1637.2019.02.010 [3] CHENG Y W, HE G, LONG Z Q. Vertical dynamic stability analysis of EDS levitation systems based on Halbach magnet arrays[C]//2009 Chinese Control and Decision Conference. Guilin: IEEE, 2009: 3726-3730. [4] 郑杰. 感应线圈对磁浮系统性能影响研究[D]. 长沙: 国防科学技术大学, 2006. [5] CHEN S S, ZHU S, CAI Y. On the unsteady-motion theory of magnetic forces for maglev[R]. Argonne : Office of Scientific and Technical Information (OSTI), 1996. [6] ZHU S, CAI Y, ROTE D M, et al. Magnetic damping for maglev[J]. Shock and Vibration, 1998, 5: 119-128. doi: 10.1155/1998/480273 [7] HAN Q H. Analysis and modeling of the EDS maglev system based on the Halbach permanent magnet array[D]. Orlando: University of Central Florida, 2004. [8] KO W. Modeling and analysis of the EDS maglev system with the Halbach magnet array[D]. Orlando: University of Central Florida, 2007. [9] HAN C, KO W, LIN K C, et al. Study of a hybrid magnet array for an electrodynamic maglev control[J]. Journal of Magnetics, 2013, 18(3): 370-374. doi: 10.4283/JMAG.2013.18.3.370 [10] 贺光. 基于Halbach结构的永磁电动与电磁混合悬浮技术研究[D]. 长沙: 国防科学技术大学, 2010. [11] LONG Z Q, HE G, XUE S. Study of EDS & EMS hybrid suspension system with permanent-magnet halbach array[J]. IEEE Transactions on Magnetics, 2011, 47(12): 4717-4724. doi: 10.1109/TMAG.2011.2159237 [12] 闫宇壮,李云钢,程虎. 电动电磁混合磁浮悬浮稳定性及技术特性分析[J]. 中国电机工程学报,2007,27(6): 53-56. doi: 10.3321/j.issn:0258-8013.2007.06.012YAN Yuzhuang, LI Yungang, CHENG Hu. Analysis of levitation stability and technology characters of EDS and EMS hybrid maglev[J]. Proceedings of the CSEE, 2007, 27(6): 53-56. doi: 10.3321/j.issn:0258-8013.2007.06.012 [13] 陈殷,张昆仑. Halbach永磁阵列空间磁场的解析计算[J]. 磁性材料及器件,2014,45(1): 1-4,9. doi: 10.3969/j.issn.1001-3830.2014.01.001CHEN Yin, ZHANG Kunlun. Analytic calculation of the magnetic field created by Halbach permanent magnets array[J]. Journal of Magnetic Materials and Devices, 2014, 45(1): 1-4,9. doi: 10.3969/j.issn.1001-3830.2014.01.001 [14] LUO C, ZHANG K L, DUAN J H, et al. Study of permanent magnet electrodynamic suspension system with a novel halbach array[J]. Journal of Electrical Engineering & Technology, 2020, 15(2): 969-977. [15] 陈殷,张昆仑. 板式双边永磁电动悬浮电磁力计算[J]. 电工技术学报,2016,31(24): 150-156.CHEN Yin, ZHANG Kunlun. Calculation of electromagnetic force of plate type null double side permanent magnet electrodynamic suspension[J]. Transactions of China Electrotechnical Society, 2016, 31(24): 150-156. [16] 雷银照. 时谐电磁场解析方法[M]. 北京: 科学出版社, 2000. [17] MELCHER J R. Continuum electromechanics[M]. Cambridge: The MIT Press, 1981. [18] KIM W J. High-precision planar magnetic levitation[D]. Cambridge: Massachusetts Institute Technology, 1997. [19] 宋玉晶,张鸣,朱煜. Halbach永磁阵列磁场解析求解及推力建模[J]. 电工技术学报,2014,29(11): 61-67. doi: 10.3969/j.issn.1000-6753.2014.11.008SONG Yujing, ZHANG Ming, ZHU Yu. Modeling of halbach array magnetic field and motor thrust[J]. Transactions of China Electrotechnical Society, 2014, 29(11): 61-67. doi: 10.3969/j.issn.1000-6753.2014.11.008 [20] 赵博, 张洪亮. Ansoft12在工程电磁场中的应用[M]. 北京: 水利水电出版社, 2010. [21] 王莉,张昆仑. 基于零功率控制策略的混合磁悬浮系统[J]. 西南交通大学学报,2005,40(5): 667-672. doi: 10.3969/j.issn.0258-2724.2005.05.022WANG Li, ZHANG Kunlun. Hybrid magnetic suspension system based on zero power control strategy[J]. Journal of Southwest Jiaotong University, 2005, 40(5): 667-672. doi: 10.3969/j.issn.0258-2724.2005.05.022 [22] 王莉. 混合EMS磁悬浮系统研究[D]. 成都: 西南交通大学, 2006. 期刊类型引用(3)
1. 王远航. 地锚拉杆对无缝线路稳定性的影响. 国防交通工程与技术. 2024(02): 22-26 . 
百度学术2. 张智海，肖宏，刘光鹏，王阳，迟义浩. 三枕捣固作业中捣镐夹持力对有砟道床作用效应影响研究. 铁道学报. 2024(10): 132-143 . 百度学术3. 祁天佑. 基于离散元的小半径曲线有砟道床横向阻力特性及加强措施研究. 机械设计与制造工程. 2022(05): 99-103 . 百度学术其他类型引用(7)
-
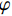
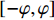
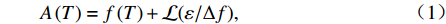
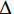
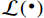
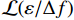
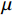
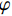
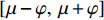
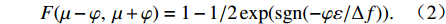
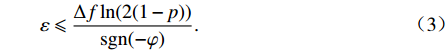
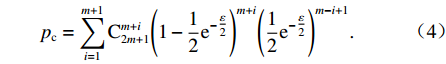
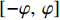
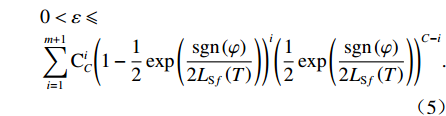
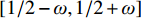
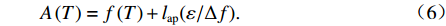
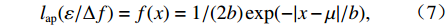
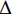
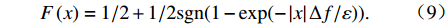
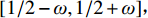
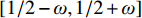
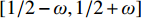
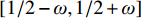
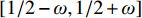
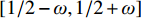
下载:
下载:
百度学术
点击查看大图
计量
- 文章访问数: 435
- HTML全文浏览量: 280
- PDF下载量: 39
- 被引次数: 10