Corrosion Detection Based on Frequency Spectrum Difference Coefficient of Higher-Order Lamb Modes
-
摘要:
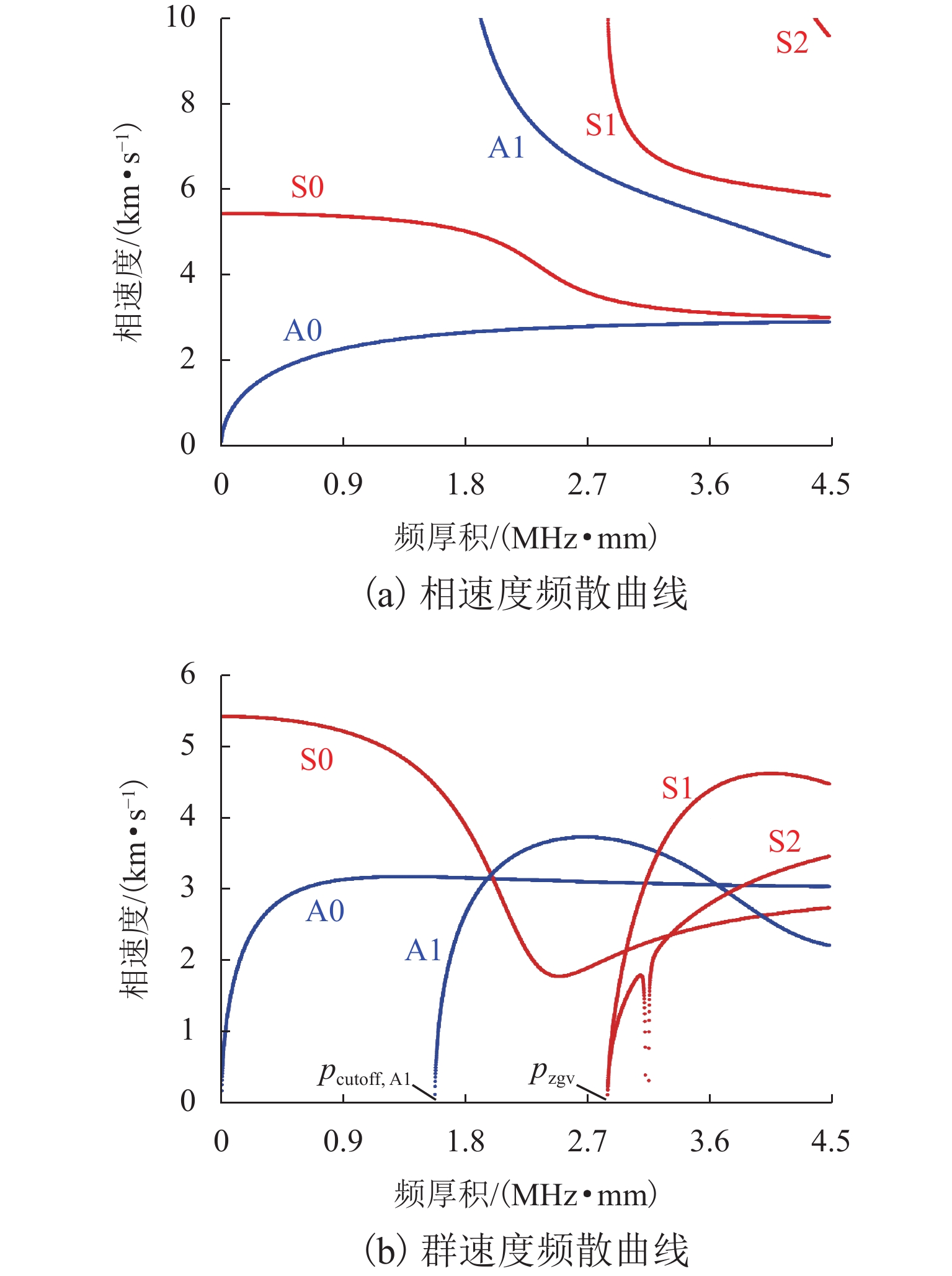
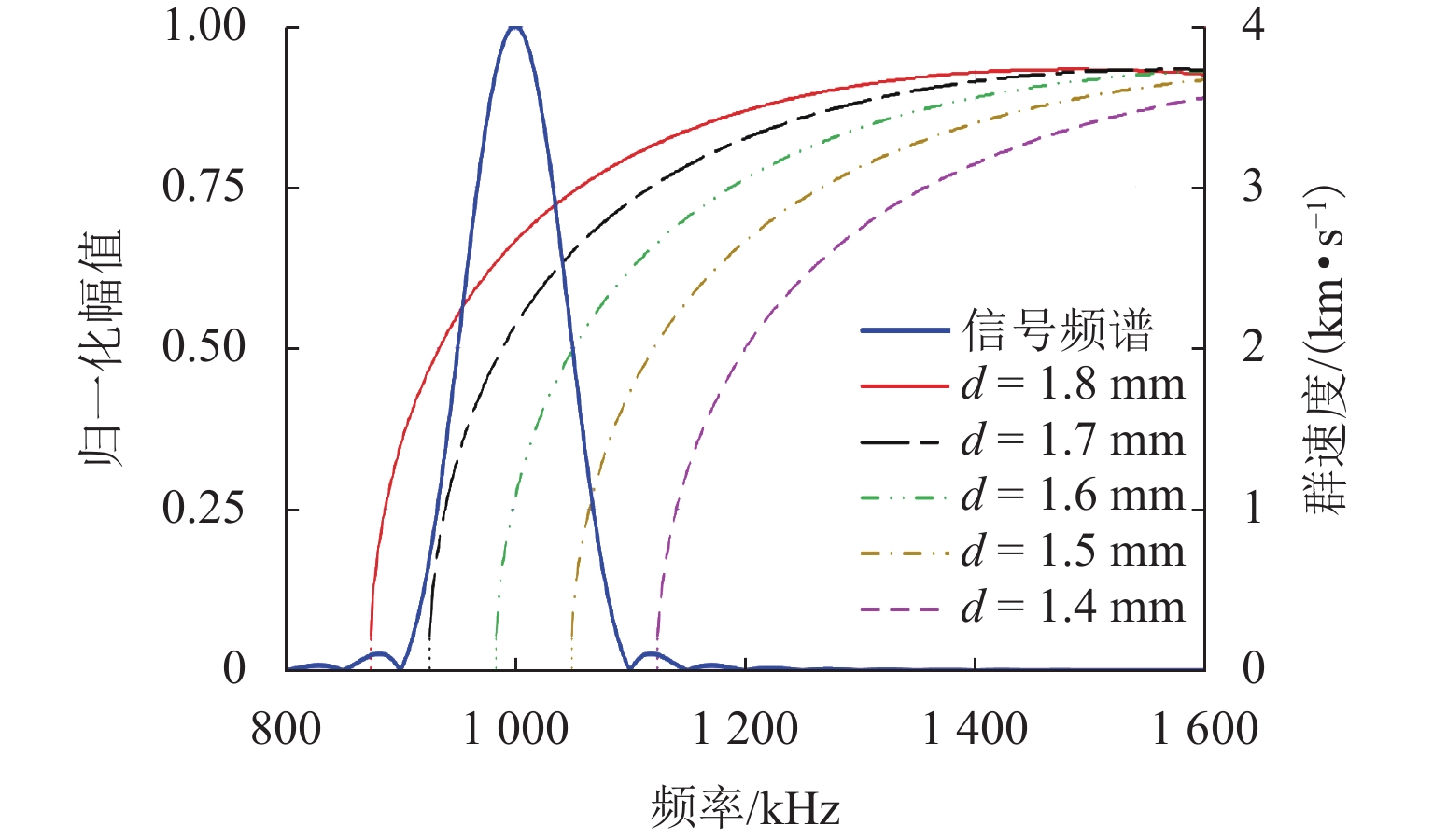
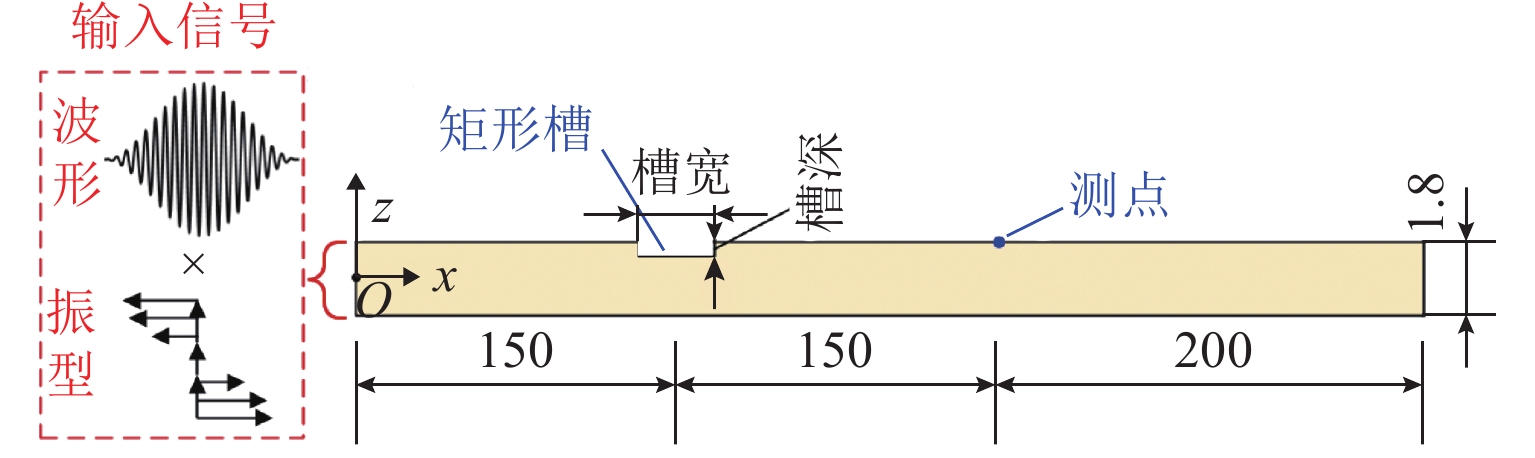
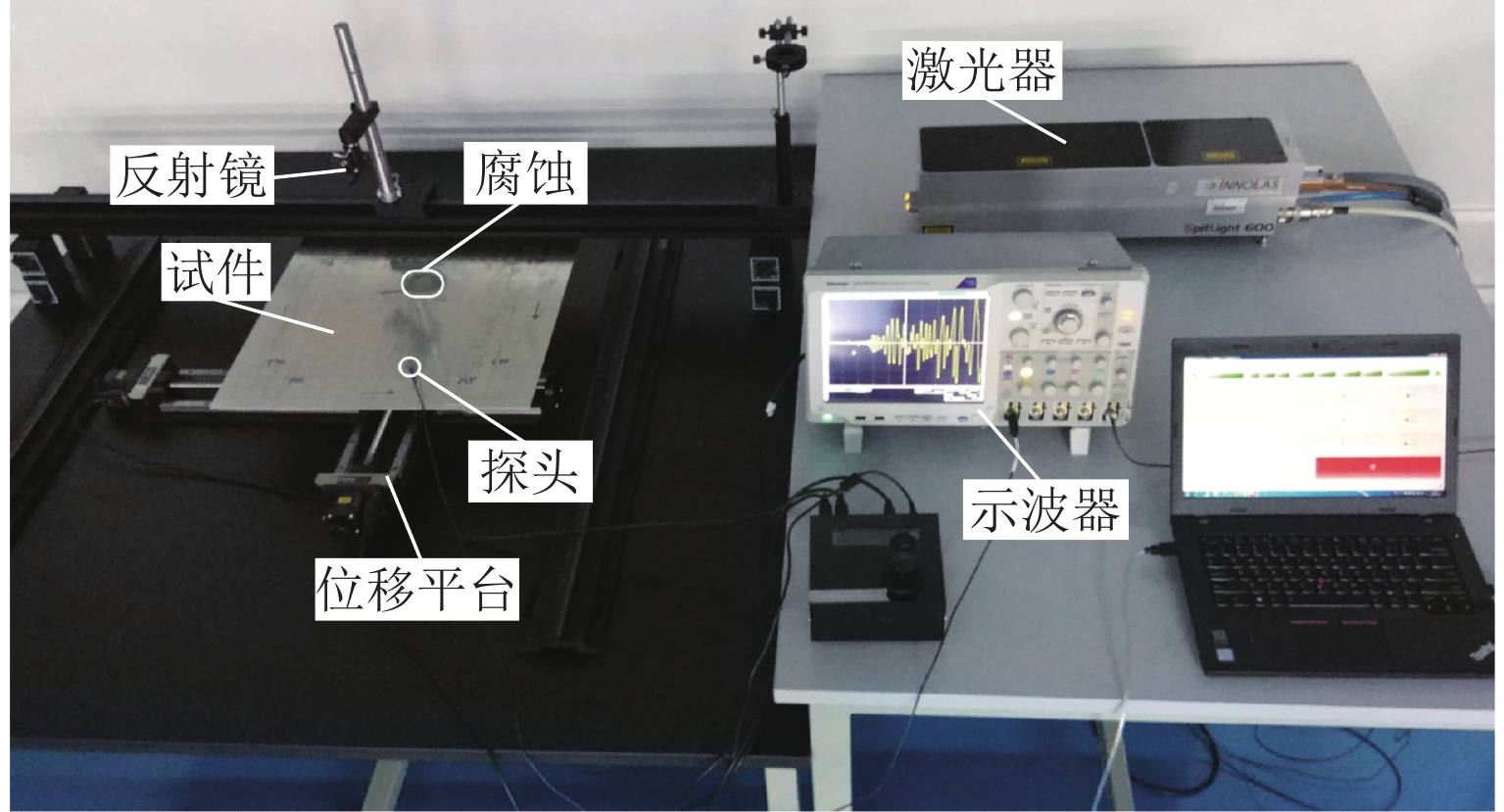
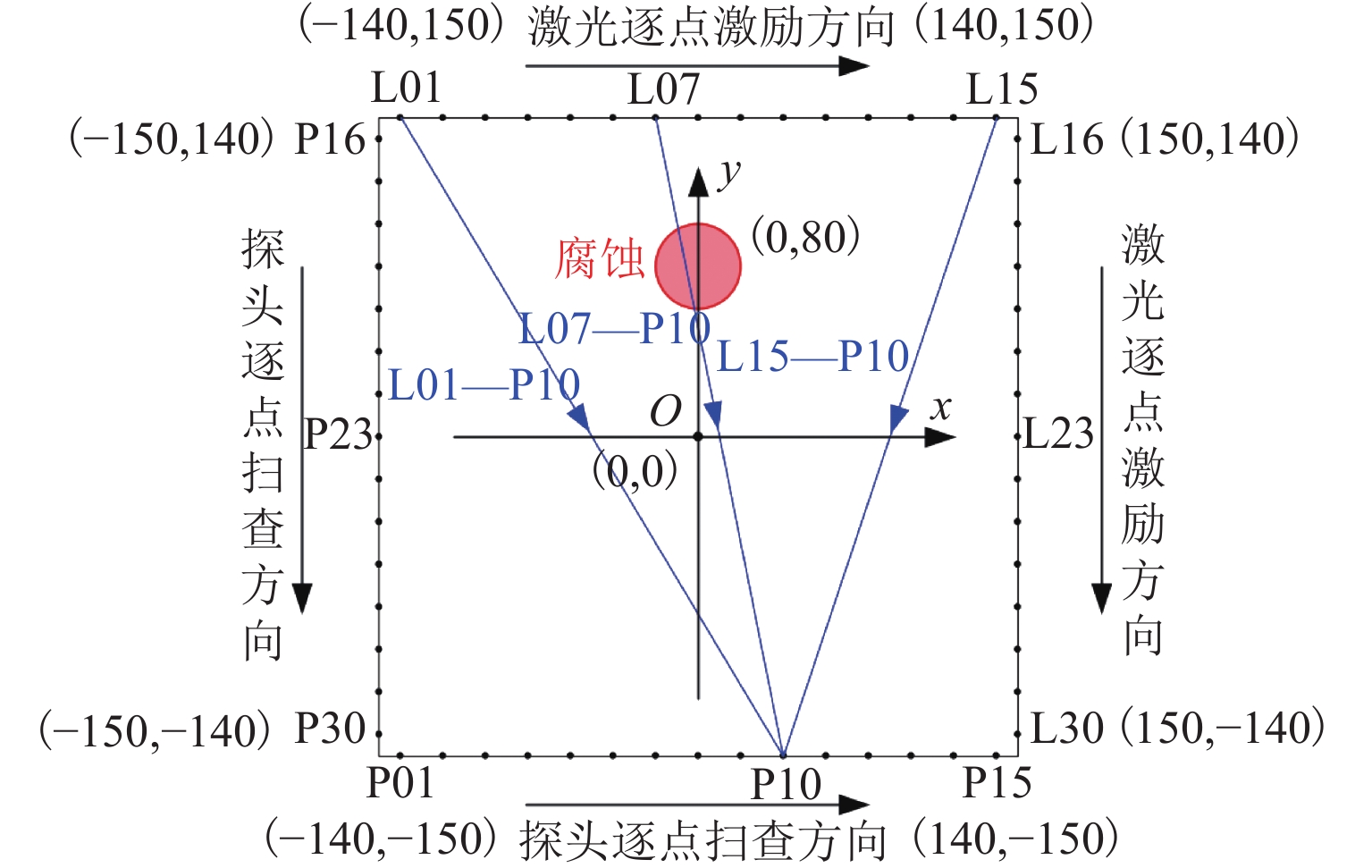
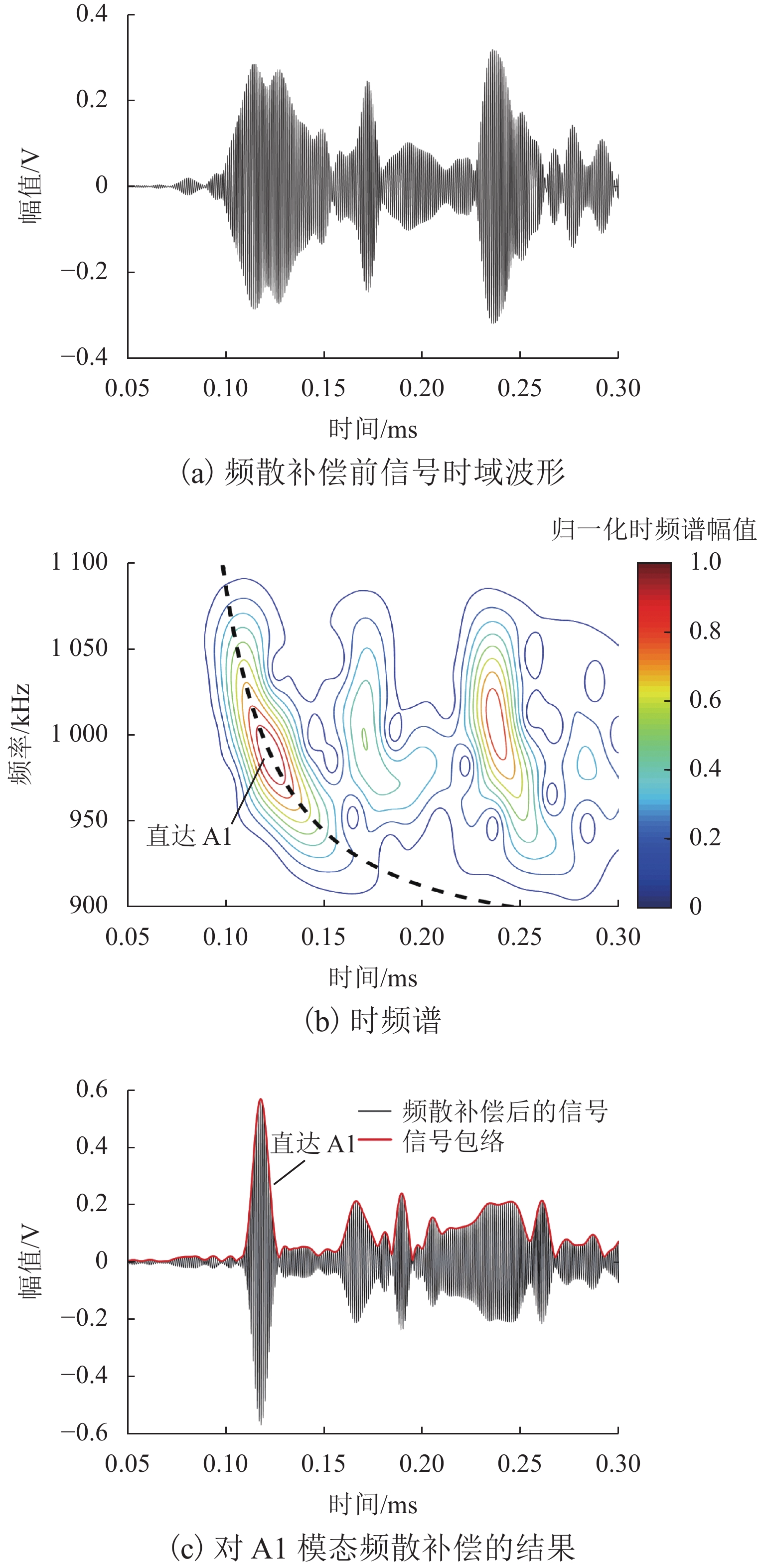
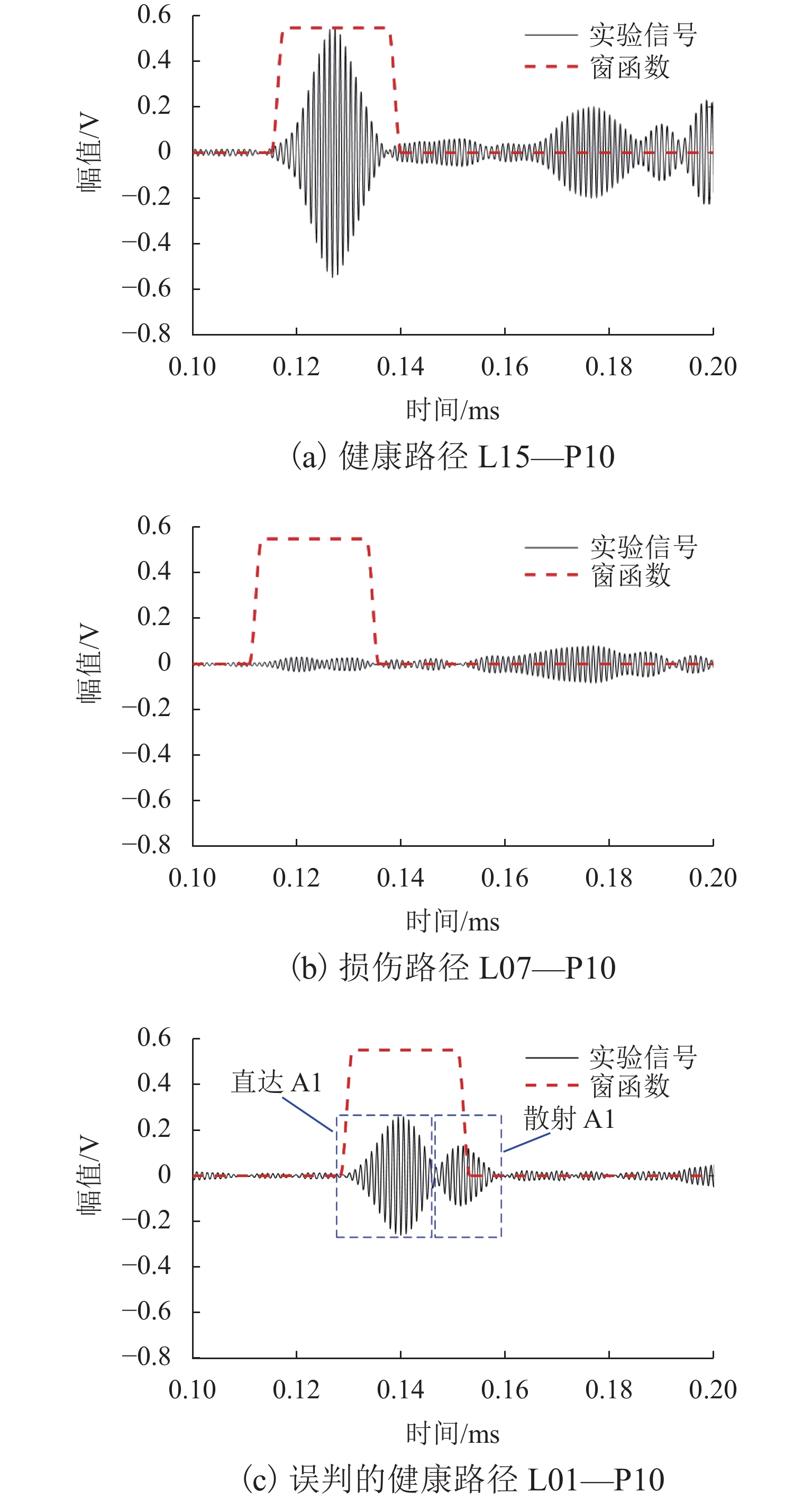
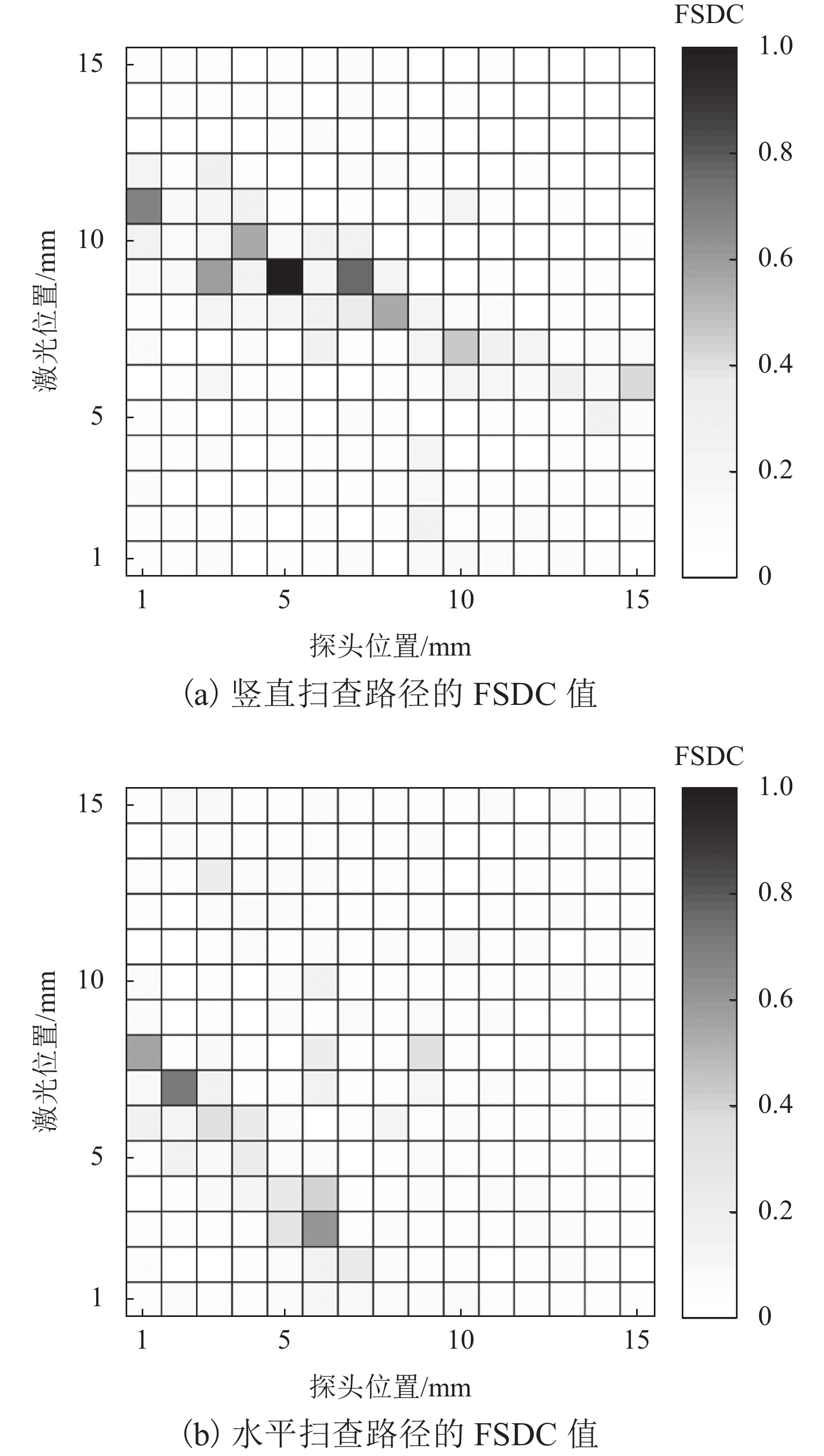
针对工业设备中大型薄壁结构件的腐蚀检测问题,提出一种基于频谱相干性分析的高阶Lamb波腐蚀损伤检测方法. 首先,利用略高于截止频率的A1模态Lamb波对含腐蚀薄壁结构的不同位置进行激励,并采集各传播路径上的响应信号;随后,采用频散补偿技术消除信号中的频散效果,通过合适的窗函数对信号中的A1模态直达波包进行分离提取,建立其与激励信号的频谱差异系数 (frequency spectrum difference coefficient, FSDC),通过有限元仿真研究该指标对不同宽度、深度腐蚀损伤的敏感性;最后,在含腐蚀铝板上进行实验验证,结合各路径FSDC指标与概率成像算法,对检测区域的腐蚀损伤进行定位与成像. 结果表明:FSDC值在健康状态下为0,而在不同宽度、深度的腐蚀影响下FSDC在0~1;相较于传统层析成像方法,所提出方法具有更好的检测灵敏度和抗干扰能力.
Abstract:In view of the corrosion detection of large thin-walled structural parts in industrial equipment, a corrosion detection method based on spectral coherence analysis using high-order Lamb waves was proposed. Firstly, the A1 mode Lamb wave with a frequency slightly higher than the cutoff frequency was adopted and transmitted at different positions on the corroded thin-walled structure, and the response signal of each propagation path was collected; then, the dispersion compensation technique was used to eliminate the dispersion effect in the signal, so the direct wave packet of A1 mode could be separated and extracted from the signal using a suitable window function. On this basis, the frequency spectrum difference coefficient (FSDC) of the extracted wave packet and the excitation signal was established as an index, which was subsequently discussed in terms of its sensitivity to corrosion defects of different widths and depths with the help of finite element simulation; finally, an experimental validation was conducted on a corroded aluminum plate, where the FSDC index of each path was combined with the probability imaging algorithm to locate and visualize the corrosion defect in the detection area. Results show that the FSDC value keeps zero for an intact path and stays between 0 and 1 for corrosions of different widths and depths. Compared with the traditional tomography method, the proposed method has better detection sensitivity and anti-interference ability.
-
Key words:
- Lamb waves /
- higher-order modes /
- cutoff property /
- corrosion /
- defect detection
-
地下结构通常被认为比地面结构具有更好的抗震性能. 然而,1995年阪神地震中神户市大量地铁车站和区间隧道的严重破坏[1-2],2008年汶川地震中约百余座隧道严重受损[3],2022年门源地震中大梁隧的严重破坏[4],2023年土耳其7.8级地震中Erkenek隧道的破坏[5]等均造成了巨大的经济损失,诸多的震害实例提醒我们应该重视地下结构的抗震问题.
目前,在地铁车站等地下结构的抗震研究中,多以设防地震作用下地下结构的动力响应特征、地震损伤机理及抗减震措施等为主[6-9]. 此类研究的共同特点是针对特定强度水平地震或针对特定的地震动记录进行的. 随着基于性能的抗震设计方法的发展,对地下结构的抗震设计不再仅限于某特定强度下结构的动力响应,而是更多地关注地下结构在不同强度地震作用下的抗震性能和损伤情况. 基于增量动力分析(IDA)方法的地下结构地震易损性分析能够得到不同强度地震作用下结构超越各性能水准的概率和发生各破坏状态的概率,能够从概率的角度定量地评价结构的抗震性能,是近年来地下结构抗震分析中被诸多学者频繁使用的一种方法[10-15].
综合分析近年来地下结构地震易损性方面的研究成果可以发现,研究对象主要以隧道和地铁车站为主. 在隧道的地震易损性研究方面,黄忠凯等[10]建立上海典型软弱场地隧道的易损性曲线,并与美国生命线联盟(ALA)指南中隧道的经验易损性曲线进行对比;刘立荣等[11]提出一种高效计算山岭隧道地震易损性的模型,基于该模型建立了某山岭隧道的地震易损性曲线;丁祖德等[12]考虑衬砌劣化对隧道抗震性能的影响,建立了考虑混凝土劣化、钢筋锈蚀条件下的隧道时变地震易损性曲线. 在地铁车站结构地震易损性研究方面,钟紫蓝等[13]建立了两层三跨地铁车站结构的地震易损性曲线,并与现有地下结构经验地震易损性曲线进行对比;许民泽等[14]考虑场地类别及地震动不确定性的影响,建立了饱和沙土场地中地铁车站结构的地震易损性曲线;陈晋男等[15]基于增量动力分析方法建立某装配式地铁车站结构的地震易损性曲线,并对不同性能状态的损伤概率进行了评估. 可以发现,目前地下结构的地震易损性研究还处于初期阶段,各学者所采用的理论和方法主要借鉴于地上结构地震易损性研究成果,在地震动强度指标选择、结构性能水准划分、不确定性因素影响考虑等方面还有待进一步提升. 另外,目前有关地下结构地震易损性研究的场地大多以典型黏土、沙土等为主,对于黄土地层地下结构的地震易损性研究较少,黄土由于其结构特性在地震作用下极易发生破坏. 因此,对黄土地层地下结构开展地震易损性研究具有重要的理论和工程意义.
本文以某黄土场地浅埋两层三跨地铁车站为例,初步探讨地下结构地震易损性分析的方法和流程,基于增量动力分析方法对该地铁车站结构进行地震易损性分析,获得不同水平地震作用下车站结构超越各性能水准的概率和发生不同破坏状态的概率,结果可为同类型地铁车站结构的易损性分析和风险评价提供参考.
1. 地下结构地震易损性分析方法
1.1 地震易损性定义
结构地震易损性是指在给定强度的地震作用下,结构达到某种破坏状态的可能性,也可以理解为结构达到或超过某种极限状态(性能水准)的概率. 地震易损性描述了地震动强度和结构损伤程度之间的关系,从概率的角度定量反映了结构的抗震性能.
相关研究表明,采用双参数对数正态分布模型建立浅埋地下结构的地震易损性曲线,具体用函数表示[16-17]为
Pf(ds⩾dsi∣IIM)=Φ(1βtotln(IIMIIMi)), (1) 式中:Pf(·)为在给定地震动强度IIM下结构地震响应ds超过某极限状态的概率;dsi为第i个极限状态对应的结构损伤限值;IIMi为第i个极限状态的结构损伤限值dsi所对应的地震动强度指标大小;Φ(·)为标准正态分布函数;βtot 为总的对数标准差,考虑了3种互相独立的不确定性影响,如式(2).
βtot=√β2ds+β2c+β2d, (2) 式中:βds为结构损伤状态定义的偏差引起的不确定性影响,取0.4[12-13,17];βc为结构形式不确定对结构承载能力的不确定性影响,取0.3[10-12,17];βd为结构动力计算结果的对数标准差.
1.2 基于IDA方法的地震易损性分析流程
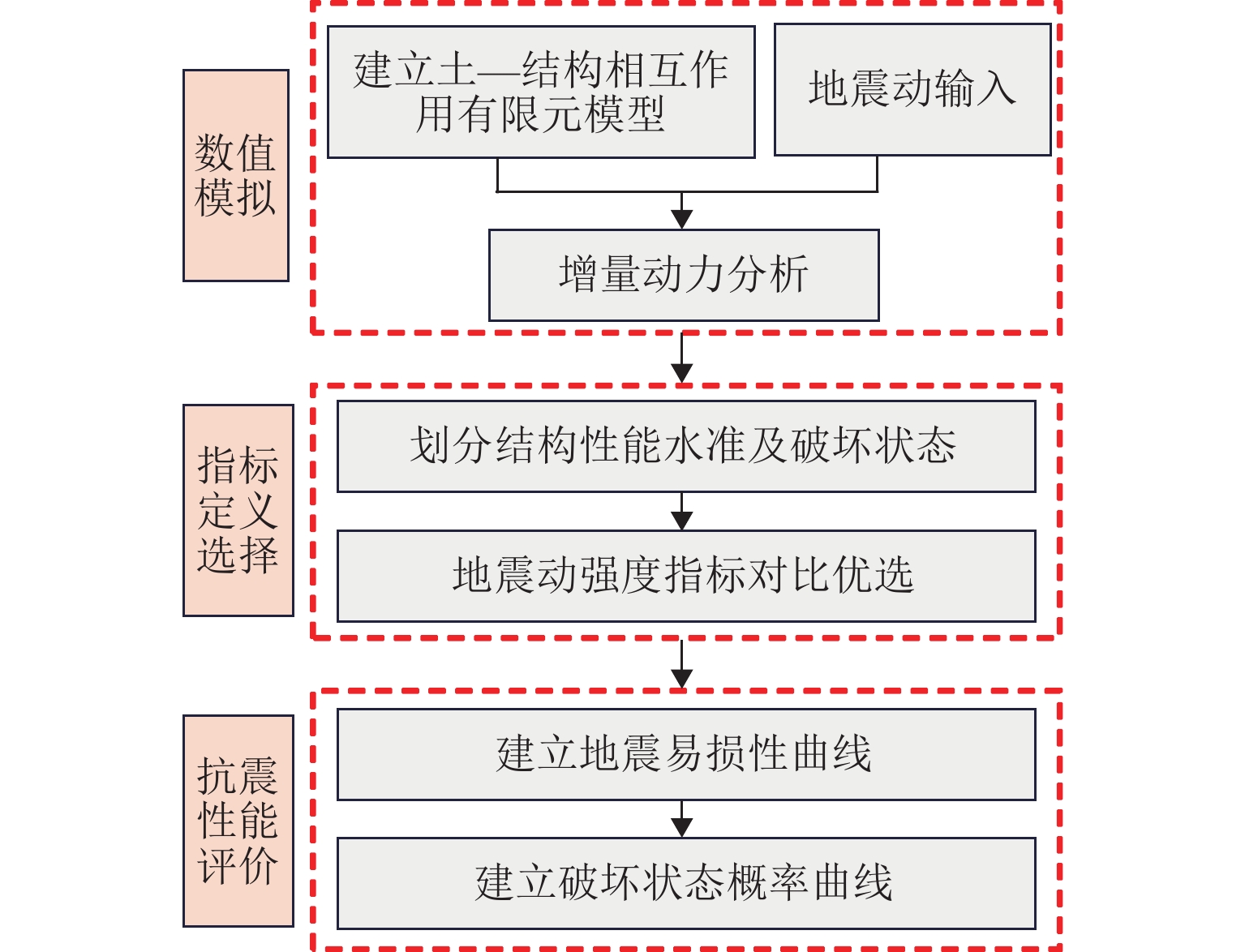
本文采用的基于IDA方法的地下结构地震易损性分析流程如图1所示. 首先,建立土-地下结构相互作用有限元模型,选择合适的地震动输入方法;其次,根据场地规范反应谱选择数条震动记录,对车站结构进行增量动力分析来获取地下结构的最大地震响应,同时划分结构的性能水准,标定结构性能水准的损伤限值,基于增量动力分析结果选择适合的地震动强度指标;最后,以结构损伤指标、地震动强度指标为基础,采用双参数对数正态分布模型建立地下结构的地震动易损性曲线和破坏状态概率曲线,来对地下结构的抗震性能进行评价.
2. 计算模型及地震动输入
2.1 计算模型
以某黄土场地两层三跨地铁车站为例进行分析,该车站为钢筋混凝土结构,高13.74 m,宽21.6 m,顶板厚度为0.8 m,中板厚度为0.4 m,底板厚度为0.9 m,侧墙宽度为0.7 m,中柱纵向长度为1.2 m,横向宽度为0.8 m,以9.12 m沿纵向等间距分布.
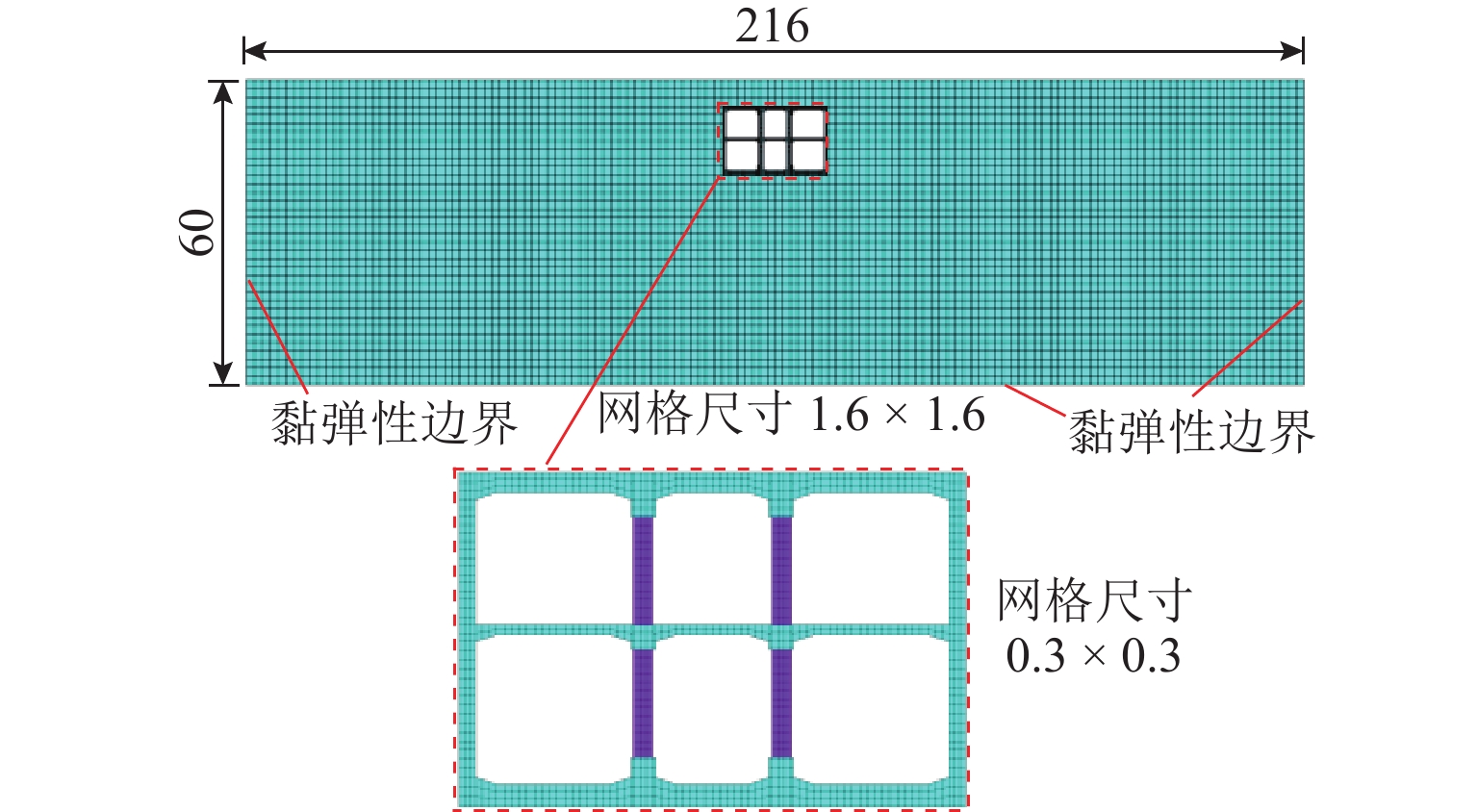
为消除边界效应,模型宽度取结构的10倍即216 m,高度取60 m,车站覆土厚度为5 m,建立土-地铁车站结构相互作用有限元模型,如图2所示. 模型两侧及底边界设置黏弹性人工边界来模拟无限域地基辐射阻尼效应和弹性恢复性能;采用面-面接触方式模拟土-地铁车站结构之间的接触行为,指定车站结构表面为目标面,土体表面为接触面,分别采用TARGE169、CONTA172单元模拟,不考虑土体-车站结构之间的张开和滑移,因此接触面行为设置为绑定. 土体和车站结构均采用二维平面应变单元模拟,同时采用理想弹塑性DP(Drucker-Prager)材料本构模型来考虑土体及结构的非线性特征[18-19]. 由于中柱不是连续分布,计算按刚度和质量不变的原则对中柱进行等效化处理,参考文献[20]中的方法对中柱的弹性模量和密度进行折减,将等间距分布的中柱等效为连续分布的纵墙. 主要计算参数如表1所示,其中,黄土参数由试验测得[18],墙、板混凝土参数按实际工程经验进行取值.
表 1 主要计算参数Table 1. Main calculation parameters材料 密度/(kg·m−3) 弹性模量/GPa 泊松比 黏聚力/kPa 内摩擦角/(°) 土体 1 650 0.07 0.30 0.03 22 墙、板 2 500 32.50 0.20 2.50 55 中柱(折减) 329 4.14 0.20 2.50 55 2.2 地震动输入
采用将地震动转化为黏弹性人工边界上等效节点荷载的方法来实现地震动的输入. 如图3所示,设时刻t作用在人工边界任一节点D处x、y方向的等效节点荷载分别为FDT(t)和FDN(t),根据人工边界处节点的运动方程,其计算式为
{FDT(t)=KDTu0(x,y,t)+CDT˙u0(x,y,t)+τ0(x,y,t),FDN(t)=KDNv0(x,y,t)+CDN˙v0(x,y,t)+σ0(x,y,t), (3) 式中:KDN、KDT分别为法向和切向的弹簧刚度系数,CDN、CDT分别为法向和切向的阻尼器阻尼系数,u0(x,y,t)和v0(x,y,t)分别为时刻t自由波场在x、y方向的位移,τ0(x,y,t)和σ0(x,y,t)分别为由位移产生的x、y方向的应力.
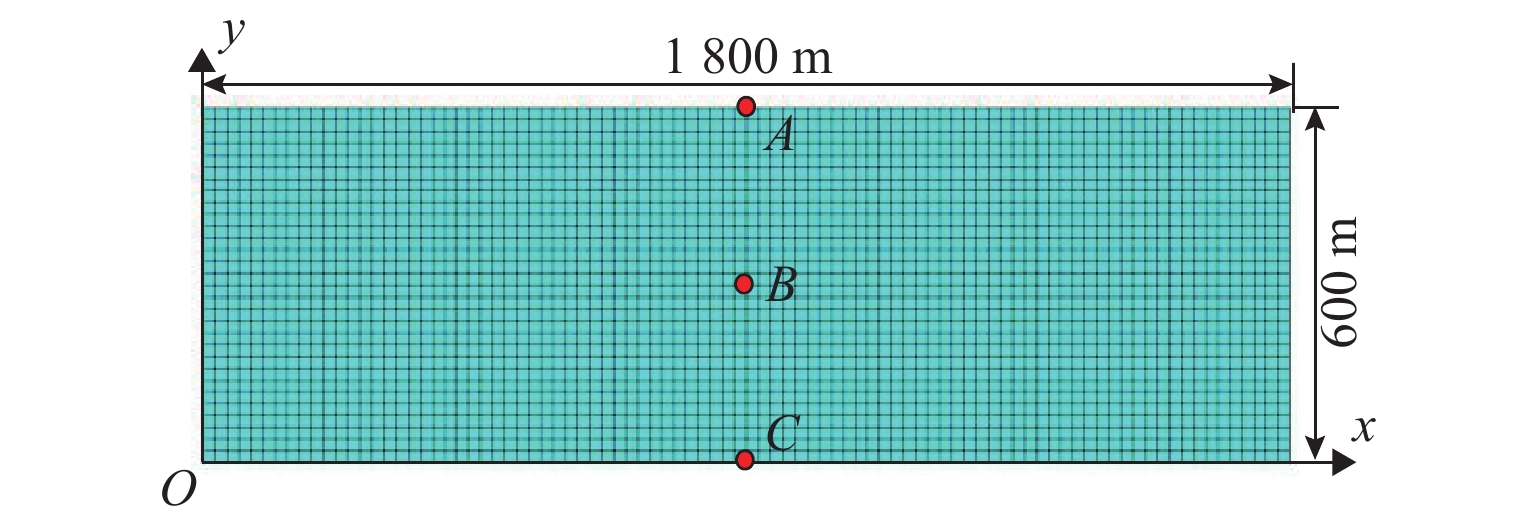
针对上述地震动输入方法,编制ANSYS-APDL程序即可实现地震动的波动输入. 为验证所编制地震波输入程序的正确性,截取一1 800 m × 600 m均匀弹性半空间模型,如图4所示. 其主要参数为:弹性模量E=1.25 GPa,泊松比μ=0.3,介质密度ρ=2 000 kg/m3. 在模型中心0 、300 、600 m处分别设置A、B、C 3个监测点. 采用脉冲波SV波垂直入射,入射波位移时程如图5所示.
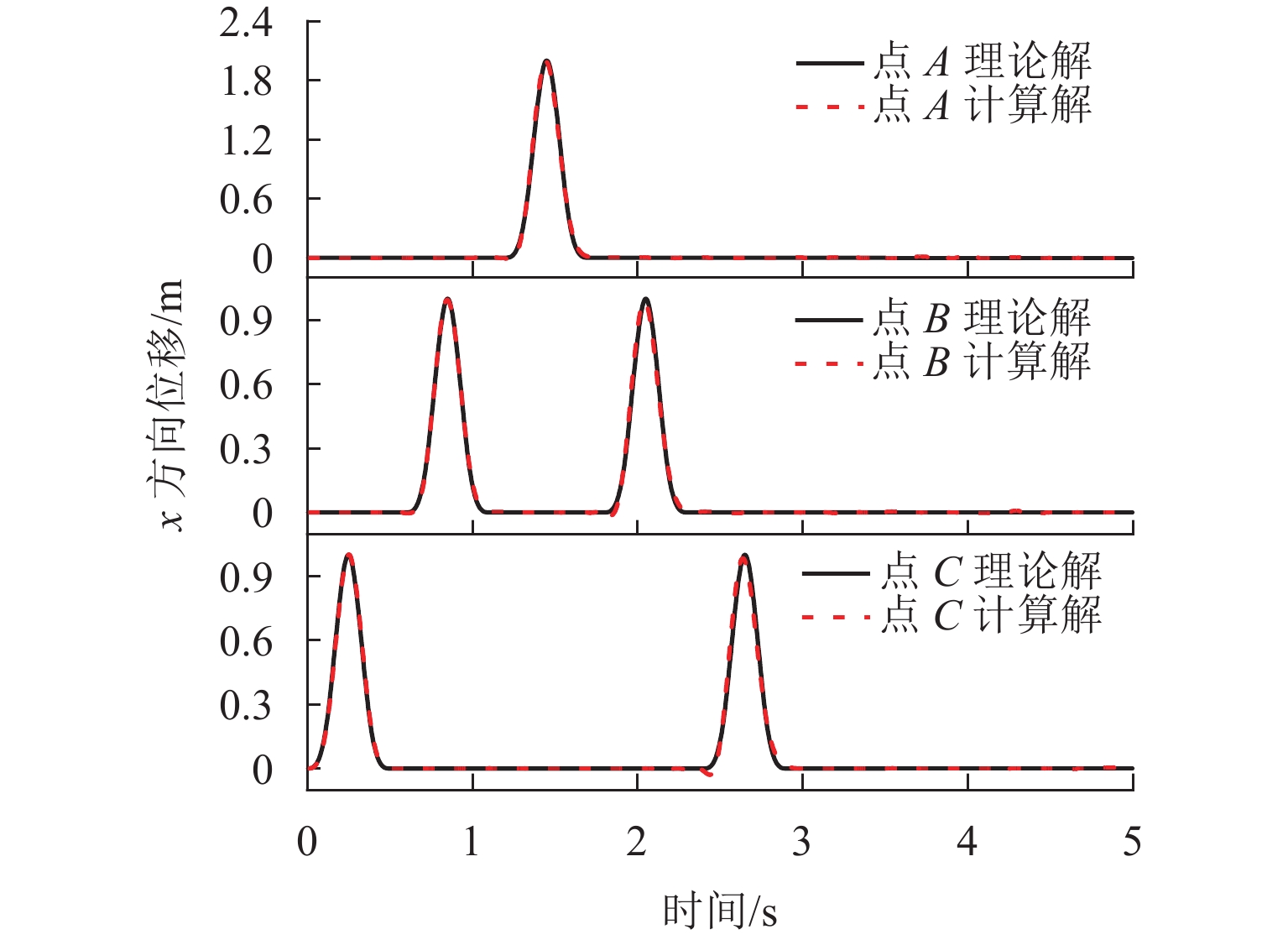
图6为监测点处的位移时程曲线. 从图中可以看出,监测点处的理论解和计算解基本吻合,从而验证了本文所采用的地震动输入方法和编制程序的正确性.
3. 增量动力分析
IDA是指将同一条地震动的幅值按比例逐级放大,对同一结构进行多次非线性动力时程分析的方法,具备静力推覆全过程非线性分析的特点,且结果都是由非线性动力时程分析结果构成,更加可靠.
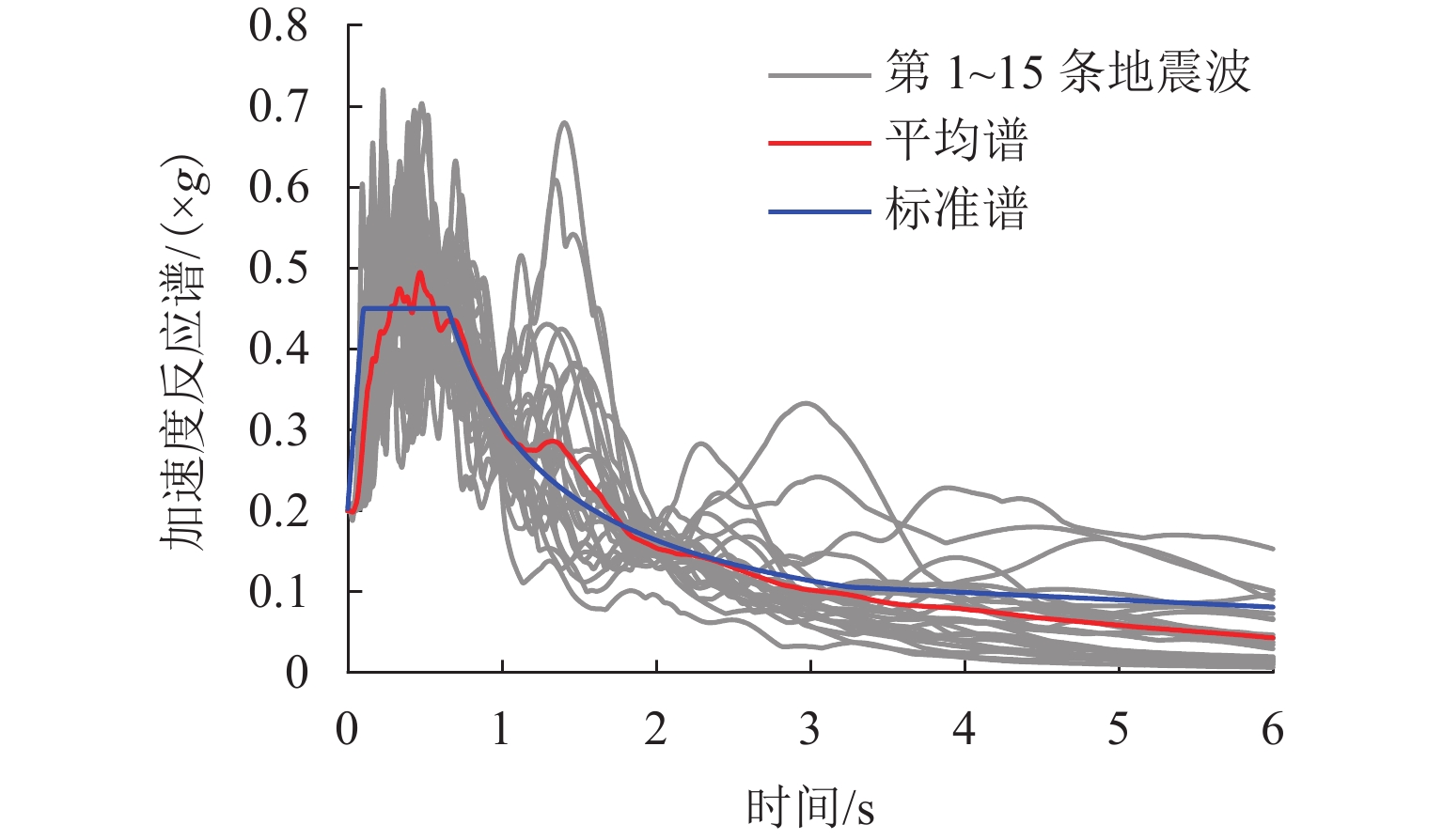
基于地震动反应谱与场地规范反应谱(8度、设防地震、Ⅲ类场地,第三组、阻尼比0.05)相匹配的方法从PEER (pacific earthquake engineering research center)强震数据库中选取15条地震动记录进行增量动力分析,图7为选取的地震动记录峰值加速度为0.2g、阻尼比为0.05时的反应谱及其平均反应谱,选择的地震动记录的平均反应谱与现行《建筑抗震设计规范》(GB 50011—2010)[21]确定的地铁车站结构所在建筑场地的规范反应谱基本吻合,且按照该规范要求两曲线在主要周期点上的误差不大于20%.
4. 结构性能水准划分
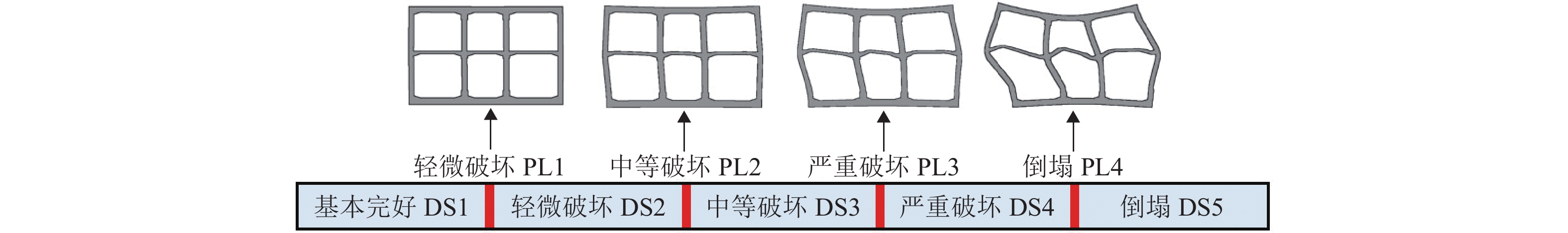
已有研究综述表明[1],地震作用下土体的水平变形对中柱产生的剪切作用是地铁车站结构发生破坏的主要原因. 因此,本文以地铁车站结构的最大层间位移角(θmax )为结构损伤指标(DDM). 将结构的性能水准(极限状态)定义为轻微破坏PL1、中等破坏PL2、严重破坏PL3、倒塌PL4四级,将地铁车站结构的破坏状态划分基本完好DS1、轻微破坏DS2、中等破坏DS3、严重破坏DS4、倒塌DS5. 性能水准与破坏状态的关系如图8所示,结构性能水准是结构破坏状态的分界,两者是不同的概念,结构处于某两个相邻的性能水准之间时,结构又可以被认为是处于某种破坏状态[22].
对各性能水准所对应的层间位移角限值,文献[23]通过地下结构Pushover分析方法对矩形框架地铁车站结构的层间位移角限值进行了标定. 结合文献[23]的研究结果,本文对地铁车站结构各性能水准功能状态的描述及其层间位移角限值标定如表2所示. 需要说明的是,对性能水准PL4的层间位移角限值按工程经验取为1%[13].
表 2 不同性能水准功能描述及损伤限值标定Table 2. Function description and damage limit calibration for different performance levels性能水准 功能状态 θmax 限值/% LS1 结构抗震关键构件基本完好,设备功能完好,可以正常运行 0.21 LS2 结构抗震关键构件发生中等损伤,设备功能整体正常,经修复后可以正常使用 0.46 LS3 结构抗震关键构件发生不可修复的严重破坏,设备功能失常,可保证安全 0.72 LS4 结构功能完全丧失,结构强度和刚度基本退化,可能发生倒塌 1.00 5. 地震动强度指标选择
地震动强度指标是表征结构所遭受的地震动强度大小的指标,合理的地震动强度指标能够反应地铁车站结构所遭受的地震动幅值、频率、持时等地震动特性. 对于相同场地中的同一个结构,采用不同的地震动强度指标来评价结构的地震响应时会存在较大的差异.
本文选取37个常用的地震动强度指标并将其分为4类,结果如表3所示. 表3中:td为地震动显著持时,可根据td=t2−t1计算,t1和t2分别为归一化Arias强度曲线中Arias强度达到总持时下Arias强度的5%和95%对应的时间;tmax为地震动总持时;n0为加速度时程曲线中单位时间内通过零点的次数;a(t)为加速度时程;v(t)为速度时程;T为周期;Sa(T,ξ)为阻尼比为ξ,周期为T时的加速度谱强度.
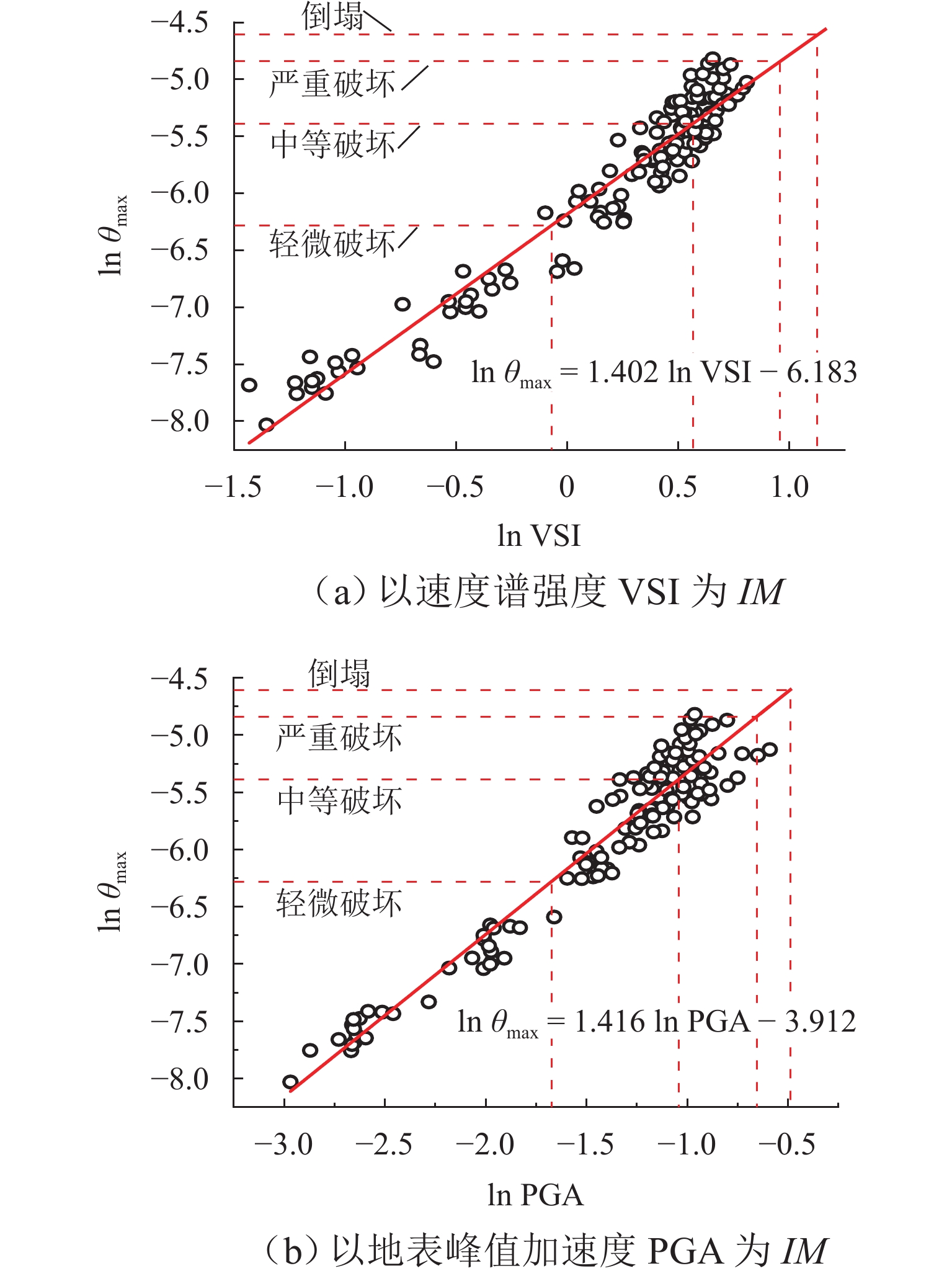
表 3 地震动强度指标选择Table 3. Selection of seismic intensity indices类型 编号 名称 定义 加速度相关型 1 地表峰值加速度 PPGA=max|a(t)| 2 加速度谱强度 AASI=∫0.50.1Sa(T,ξ)dT 3 加速度平方积分 Ea=∫tmax0a2(t)dt 4 根平方加速度 Ars=√∫tmax0a2(t)dt 5 Housner 加速度强度 Pa=1td∫t2t1a2(t)dt 6 Housner 均方根加速度 Arms=√1td∫t2t1a2(t)dt 7 地震动均方根加速度 arms=√1tmax∫tmax0a2(t)dt 速度相关型 8 累计绝对速度 CCAV=∫tmax0|a(t)|dt 9 谱加速度峰值 PPSA=maxSa(T,ξ=0.05) 10 Arias 强度 AAI=π2g∫tmax0a2(t)dt 11 修正的 Arias 强度 IAM=AAI/n20 12 特征强度 Ic=A1.5rmst0.5d 13 复合加速度 Ia=PPGAt1/3d 14 Faifar 强度 If=PPGVt0.25d 15 地表峰值速度 PPGV=max|v(t)| 16 速度谱强度 VVSI=∫2.50.1Sv(T,ξ)dT 17 速度平方积分 Ev=∫tmax0v2(t)dt 18 根平方速度 Vrs=√∫tmax0v2(t)dt 位移相关型 19 Housner 速度强度 Pv=1td∫t2t1v2(t)dt 20 Housner 均方根速度 Vrms=√1td∫t2t1v2(t)dt 21 地震动均方根速度 vrms=√1tmax∫tmax0v2(t)dt 22 累计绝对位移 CCAD=∫tmax0|v(t)|dt 23 谱速度峰值 PPSV=max(Sv(T,ξ=0.05)) 24 Housner 强度 SSI=∫2.50.1PPSV(T,ξ=0.05)dT 25 复合速度 Iv=P2/3PGVt1/3d 26 地表峰值位移 PPGD=max|d(t)| 27 位移谱强度 DDSI=∫4.02.5Sd(T,ξ)dT 28 位移平方积分 Ed=∫tmax0d2(t)dt 29 根平方位移 Drs=√∫tmax0d2(t)dt 30 Housner 位移强度 Pd=1td∫t2t1d2(t)dt 31 Housner 均方根位移 Drms=√1td∫t2t1d2(t)dt 32 地震动均方根位移 drms=√1tmax∫tmax0d2(t)dt 33 累计绝对能量 CCAI=∫tmax0|d(t)|dt 34 谱位移峰值 PPSD=maxSd(T,ξ=0.05) 35 复合位移 Id=PPSDt1/3d 比值型 36 峰值速度与峰值加速度比值 F1=PPGV/PPGA 37 峰值位移与峰值速度比值 F2=PPGD/PPGV 以最大层间位移角θmax为结构损伤指标DDM,以上述37个常见参数为地震动强度指标IIM,对增量动力分析结果进行回归分析,结果如图9所示. 限于篇幅,此处仅列出以VVSI和PPGA为地震动强度指标的拟合结果. 根据回归分析结果分别从有效性、实用性和效益性3个方面评价上述地震动强度指标的优劣,以此选择适合本文地铁车站的地震动强度指标.
5.1 有效性
地震动强度指标的有效性指在相同的地震动强度条件下,能够使结构损伤指标DDM的离散性最小,从而在不降低精度的前提下减少非线性动力时程分析的次数. 有效性可通过ln IIM与ln DDM经验拟合式的对数标准差βd进行判断[24],如式(4)
βd=√n∑j=1[lnDDM−(lna+blnIIM)]2n−2, (4) 式中:a、b为线性拟合的系数,n为非线性动力时程分析的总次数,j为分析次数.
在相同条件下,βd的值越小,所选择的地震动强度指标IIM的有效性越高,增量动力分析计算结果的离散性越小,反之亦然.
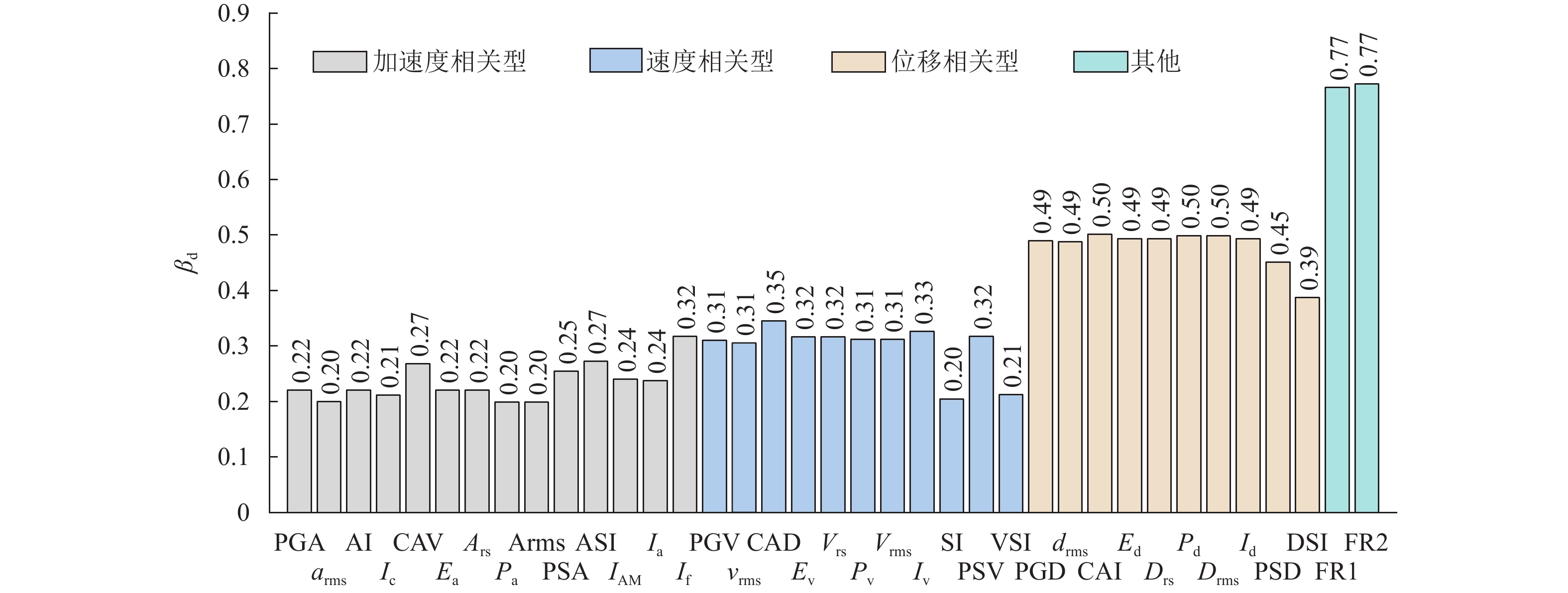
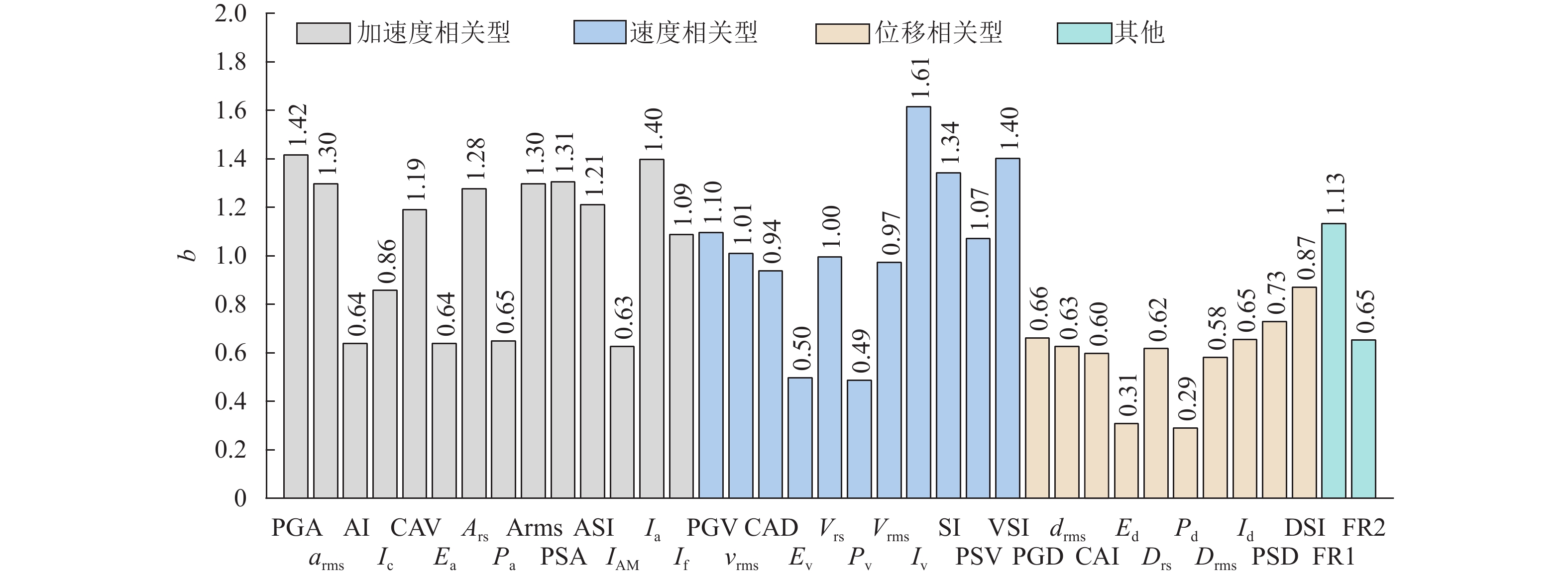
图10为不同地震动强度指标的有效性βd的对比结果. 可以发现,4 类指标的有效性从左到右整体上逐渐增大,加速度相关型中arms、Pa、Arms及速度相关型指标中SSI的有效性最高;位移相关型指标和比值型指标的离散性普遍较大,不适合作为地震动强度指标.
5.2 实用性
地震动强度指标的实用性是指结构损伤指标DDM受地震动强度指标IIM变化的影响程度大小. 实用性根据经验拟合式的系数b进行判断,b值越大,实用性越高,说明结构损伤指标DDM受地震动强度指标IIM变化的影响越大.
图11为不同地震动强度指标实用性b的对比结果. 可以发现,以层间位移角为结构损伤指标时,4类指标的有效性差距较为明显,其中Iv、PPGA、Ia、VVSI的实用性最高,Pd和Ed的实用性最低.
5.3 效益性
单独使用有效性或实用性选择地震动强度指标具有片面性,会导致选择的地震动强度存在偏差,因此,可采用效益性ζ对2个评判标准进行平衡,效益性是对有效性和实用性的综合评价,可采用式(5)计算[24].
ζ=βdb. (5) 由式(5)可知:ζ越小,选择的地震动强度指标的效益性越高.
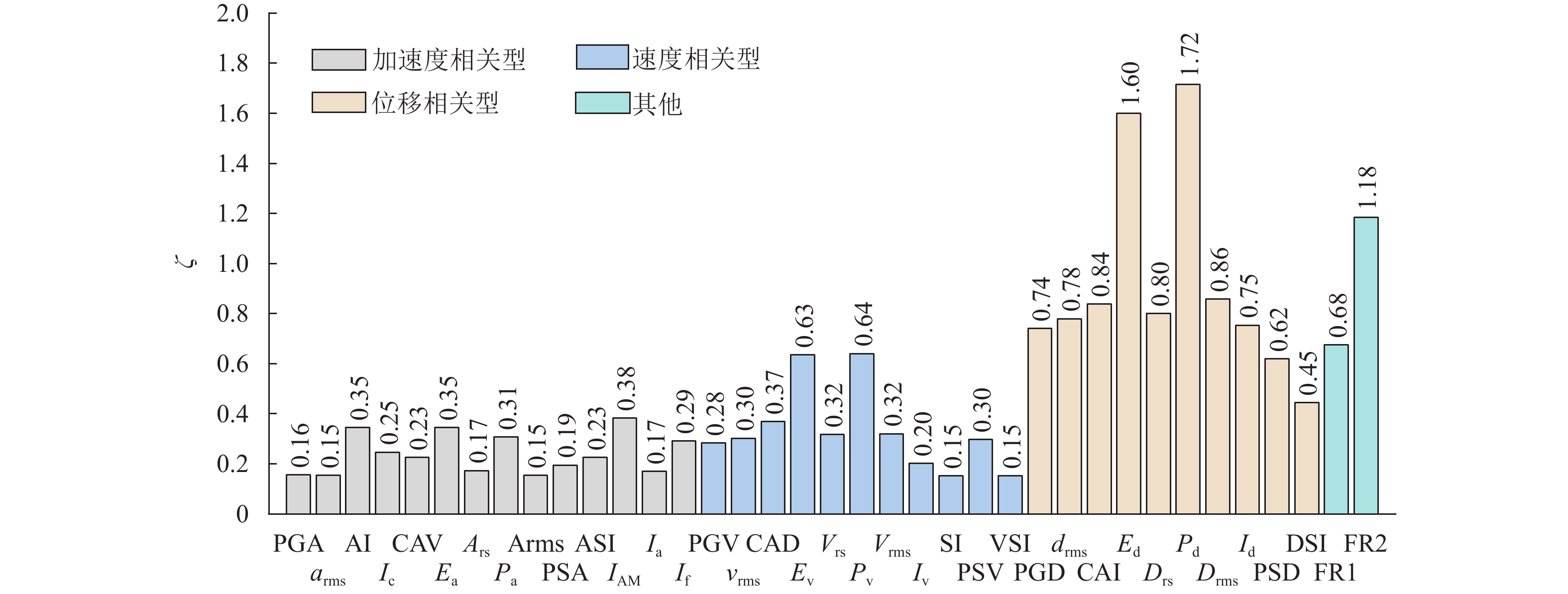
图12为不同地震动强度指标效益性ζ的对比结果. 可以发现,加速度相关型、速度相关型指标的效益性高于位移相关型指标、比值型指标;效益性最高的5个指标依次是VVSI、SSI、Arms、arms、PPGA,效益性最差的为Ed、Pd和F2.
综上所述,本文采用VVSI作为地震动强度指标进行地震易损性分析. 同时,鉴于我国现行相关抗震设计规范[21,25]中多采用PPGA表征地震动强度大小,为便于对比,此处也给出了以PPGA为地震动强度指标的分析结果.
6. 地震易损性分析
6.1 易损性曲线
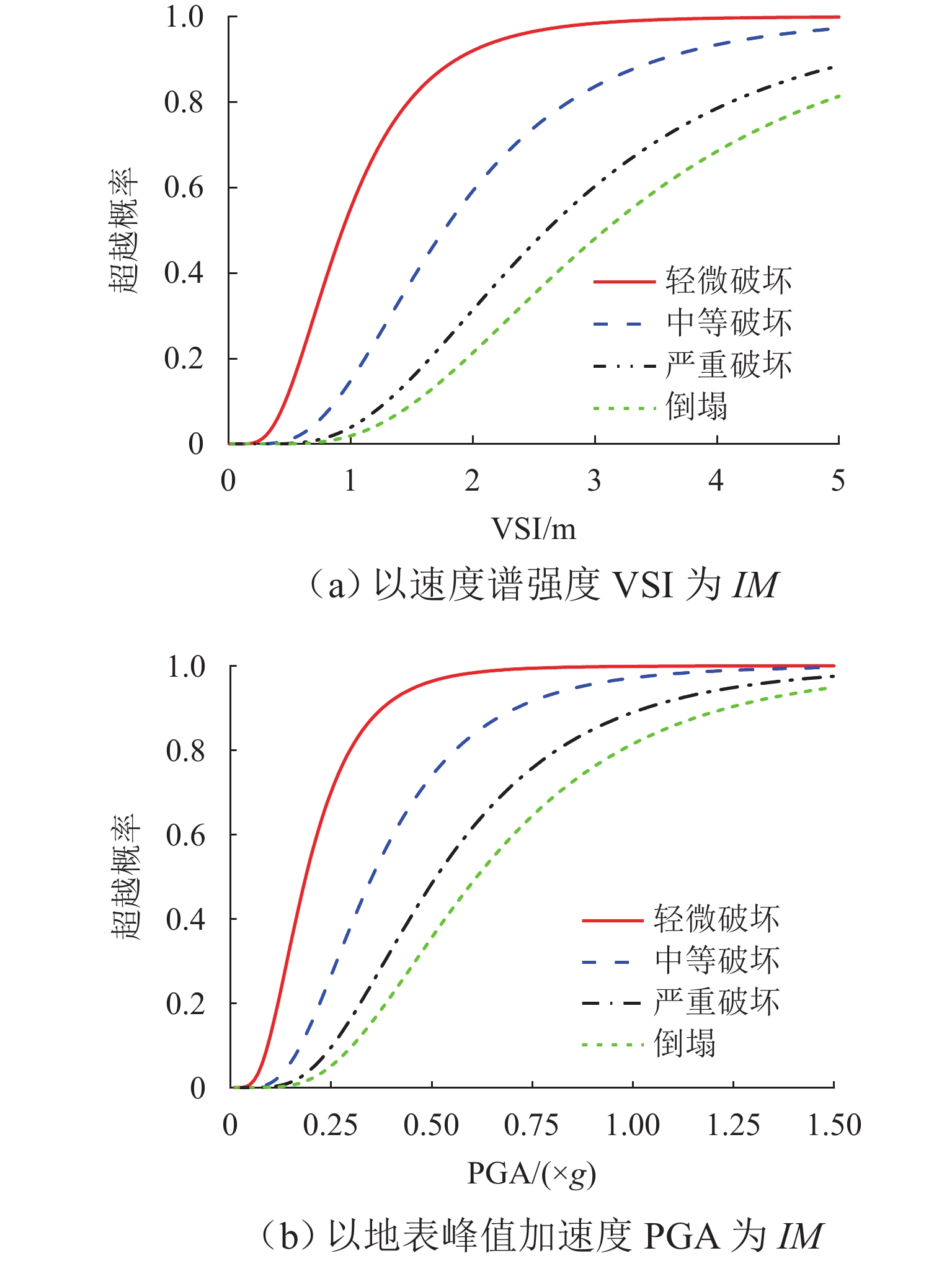
将各性能水准(极限状态)层间位移角限值dsi代入如图9所示的ln IIM与ln DDM经验拟合式中,即可求得IIMi值. 以VVSI为地震动强度指标时,4级性能水准的IIMi值分别为0.932、1.763、2.604、3.081,总的对数标准差βtot为0.543. 以PPGA为地震动强度指标时,4级性能水准的IIMi值分别为0.188、0.352、0.519、0.613,总的对数标准差βtot为0.547. 将求得的βtot和IIMi代入式(1)中,即可得到该地铁车站结构的地震易损性曲线,结果如图13所示.
从图13可以看出,随着地震动强度的增大,结构达到各破坏状态的概率逐渐趋于100%,在同一地震动强度下,结构达到倒塌的概率<严重破坏的概率<中等破坏的概率<轻微破坏的概率. 该地铁车站结构在超越概率为50%时轻微破坏、中等破坏、严重破坏及倒塌对应的VVSI值分别为0.94、1.77、2.61、3.08 m,对应的PPGA值分别为0.19g、0.36g、0.52g、0.63g.
6.2 破坏状态曲线
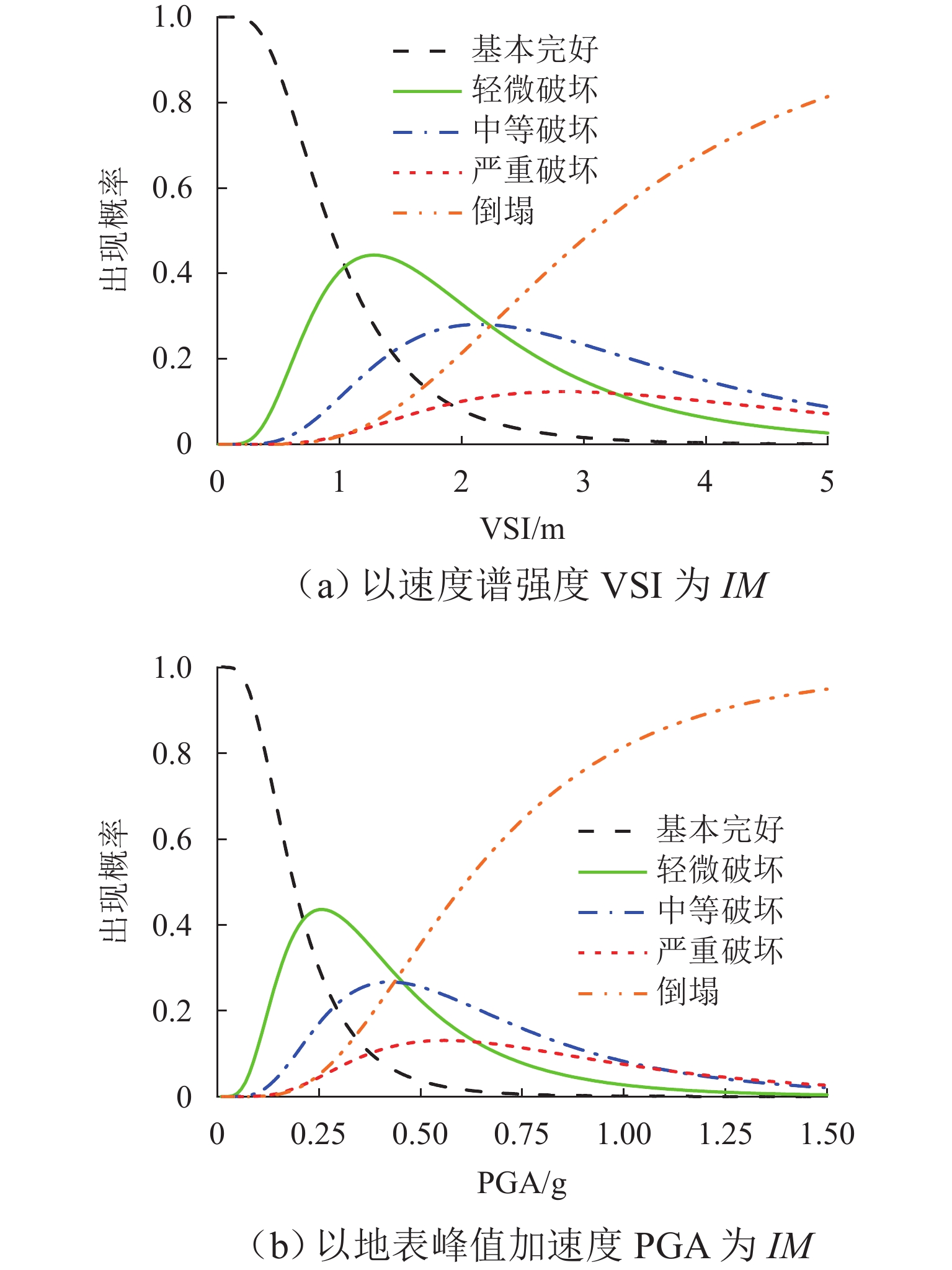
由结构性能水准和破坏状态的关系[22],可以按式(6)计算得到结构的破坏状态概率. 绘制的破坏状态概率曲线如图14所示. 从图中可以直观得到某一强度地震动下各破坏状态的出现概率.
Pf|DSj={1−Pf|LS1,j=1Pf|LSj−1−Pf|LSj,j=2,3,4Pf|LS4,j=5 (6) 表4、5分别给出了我国现行《建筑抗震设计规范》(GB 50011—2010)[21]中规定的不同频遇地震作用下各性能水准的超越概率和各破坏状态的出现概率,结果可用于同类型场地浅埋地铁车站结构的地震风险评估.
表 4 不同频遇地震各性能水准超越概率Table 4. Exceedance probability of each performance level for earthquakes with different frequencies地震频遇 设计基本加速度/(×g) 超越概率/% PL1 PL2 PL3 PL4 多遇地震 0.07 3.58 0.16 0.01 0.00 设防地震 0.20 54.70 15.20 4.29 2.03 罕遇地震 0.41 92.37 60.90 34.26 23.14 表 5 不同频遇地震各破坏状态出现概率Table 5. Occurrence probability of each damage state in earthquake with different frequencies地震频遇 设计基本加速度(×g) 出现概率/% DS1 DS2 DS3 DS4 DS5 多遇地震 0.07 96.42 3.43 0.14 0.01 0 设防地震 0.20 45.30 39.68 10.73 2.26 2.03 罕遇地震 0.41 7.63 31.47 26.44 11.12 23.14 7. 结 论
本文对某两层三跨地铁车站结构进行了增量动力分析,选择了37个地震动强度指标,并对其优劣进行了综合评价,得到了适用于本文黄土场地条件和车站结构断面形式的地震动强度指标,并以此建立该地铁车站结构的地震易损性曲线和破坏状态概率曲线,得到以下主要结论:
1) 加速度相关型、速度相关型指标更适合作为地震动强度指标来预测地下结构的地震响应,位移相关型、比值型指标不宜作为地震动强度指标.
2) 本文场地条件下车站结构在超越概率为50%时轻微破坏、中等破坏、严重破坏及倒塌对应的VVSI值分别为0.94、1.77、2.61、3.08 m,对应的PPGA值分别为0.19g、0.36g、0.52g、0.63g.
3)多遇地震作用下该车站结构发生破坏的概率较小,设防地震作用下该车站结构以轻微破坏为主,罕遇地震作用下该车站结构以轻微破坏和中等破坏为主.
致谢:兰州交通大学天佑青年人才托举工程项目(1520260306)的资助.
-
-
[1] NAGATA Y, HUANG J, ACHENBACH J D, et al. Lamb wave tomography using laser-based ultrasonics[M]. Review of Progress in Quantitative Nondestructive Evaluation. Boston: Springer, 1995: 561-568. [2] PEI J, YOUSUF M I, DEGERTEKIN F L, et al. Lamb wave tomography and its application in pipe erosion/corrosion monitoring[J]. Research in Nondestructive Evaluation, 1996, 8(4): 189-197. doi: 10.1080/09349849609409599 [3] MALYARENKO E V, HINDERS M K. Fan beam and double crosshole Lamb wave tomography for mapping flaws in aging aircraft structures[J]. The Journal of the Acoustical Society of America, 2000, 108(4): 1631-1639. doi: 10.1121/1.1289663 [4] MALYARENKO E V, HINDERS M K. Ultrasonic Lamb wave diffraction tomography[J]. Ultrasonics, 2001, 39(4): 269-281. doi: 10.1016/S0041-624X(01)00055-5 [5] BELANGER P, CAWLEY P. Feasibility of low frequency straight-ray guided wave tomography[J]. NDT & E International, 2009, 42(2): 113-119. [6] BELANGER P, CAWLEY P. Lamb wave tomography to evaluate the maximum depth of corrosion patches[C]//34th Annual Review of Progress in Quantitative Nondestructive Evaluation. Colorado: American Institute of Physics, 2008, 975(1): 1290-1297. [7] HUTHWAITE P. Improving accuracy through density correction in guided wave tomography[J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2016, 472(2186): 20150832.1-20150832.25. [8] RAO J, RATASSEPP M, FAN Z. Guided wave tomography based on full waveform inversion[J]. IEEE Transactions on Ultrasonics, Ferroelectrics, and Frequency Control, 2016, 63(5): 737-745. doi: 10.1109/TUFFC.2016.2536144 [9] RAO J, RATASSEPP M, FAN Z. Investigation of the reconstruction accuracy of guided wave tomography using full waveform inversion[J]. Journal of Sound and Vibration, 2017, 400: 317-328. doi: 10.1016/j.jsv.2017.04.017 [10] ROSE J L, BARSHINGER J N. Using ultrasonic guided wave mode cutoff for corrosion detection and classification[C]//1998 IEEE Ultrasonics Symposium. Sendai: IEEE, 1998(1): 851-854. [11] LUO Z, ZENG L, LIN J. A hidden corrosion detection method based on robust multimodal Lamb waves[J]. Measurement Science and Technology, 2020, 31(4): 044002.1-044002.12. [12] CAO X, ZENG L, LIN J, et al. A correlation-based approach to corrosion detection with Lamb wave mode cutoff[J]. Journal of Nondestructive Evaluation, 2019, 38(87): 1-16. [13] ROSE J L. Ultrasonic guided waves in solid media[M]. New York: Cambridge University Press, 2014: 104-106 [14] ZENG L, LIN J, BAO J, et al. Spatial resolution improvement for Lamb wave-based damage detection using frequency dependency compensation[J]. Journal of Sound and Vibration, 2017, 394: 130-145. [15] AULD B A. Acoustic fields and waves in solids: volume 2[M]. Florida: [s.n.], 1973: 75-94. [16] PAVLAKOVIC B N. Leaky guided ultrasonic waves in NDT[D]. London, UK: Imperial College London, 1998. [17] MICHAELS J E, LEE S J, CROXFORD A J, et al. Chirp excitation of ultrasonic guided waves[J]. Ultrasonics, 2013, 53(1): 265-270. doi: 10.1016/j.ultras.2012.06.010 [18] ZHOU C, SU Z, CHENG L. Probability-based diagnostic imaging using hybrid features extracted from ultrasonic Lamb wave signals[J]. Smart Materials and Structures, 2011, 20(12): 125005.1-125005.14. [19] SUBBARAO P M V, MUNSHI P, MURALIDHAR K. Performance of iterative tomographic algorithms applied to non-destructive evaluation with limited data[J]. NDT & E International, 1997, 30(6): 359-370. [20] HANSEN P C, JØRGENSEN J S. AIR tools II: algebraic iterative reconstruction methods, improved implementation[J]. Numerical Algorithms, 2018, 79(1): 107-137. doi: 10.1007/s11075-017-0430-x 期刊类型引用(4)
1. 谢铠泽,代玮武,李义强,杨观星,朱学伟. 插入道岔施工对运营高速道岔锁定轨温变化影响试验. 北京交通大学学报. 2024(01): 66-73 .  百度学术
百度学术2. 崔万里. 高速铁路无缝线路钢轨纵向应力与轨温监测系统研究. 现代信息科技. 2023(03): 168-171 .  百度学术
百度学术3. 郑星,凌明祥,刘谦,严侠. 压电陶瓷驱动器菱形位移放大机构的动态应变测量. 中国测试. 2019(01): 56-59 .  百度学术
百度学术4. Ganzhong Liu,Hao Liu,Anqi Wei,Jieling Xiao,Ping Wang,Shaozheng Li. A new device for stress monitoring in continuously welded rails using bi-directional strain method. Journal of Modern Transportation. 2018(03): 179-188 .  必应学术
必应学术其他类型引用(3)
-






 下载:
下载:













 下载:
下载:



 百度学术
百度学术