Spherical Layer Sampling Method for Probability Evaluation on Structural Failure
-
摘要:
传统Monte Carlo抽样方法应用于小失效概率等复杂可靠度问题时，存在效率低下、精度有限等不足，针对这一问题提出一种球层抽样分析方法. 首先，通过分离距离与方向参量，对标准正态随机向量进行重构，并验证其标准正态性与相互独立性；然后，采用分层抽样策略，将半径大于一阶可靠度指标外的正态空间划分为多个球层，进而利用所重构的向量逐层进行抽样，并结合全概率公式，形成估计结构失效概率的球层抽样算法；最后，通过对3个典型算例进行对比分析，验证算法性能. 分析表明：所提出算法具有较高的抽样效率与收敛性能，算例计算结果误差在3%以内；与其他算法相比，其估计方差更小，且可有效解决多设计验算点等复杂可靠度问题；算法在抽样效率、适用范围以及稳定性等方面具有优势，更适用于实际复杂结构可靠度的求解分析.
Abstract:When the traditional Monte Carlo sampling method is applied to complex reliability problems such as small failure probability, there are some shortcomings such as low efficiency and limited accuracy. To solve this problem, a spherical layer sampling analysis method is developed. Firstly, by dividing the distance and direction parameters, the standard normal random vector is reconstructed, and its standard normality and mutual independence are verified. Thereafter, based on a layered sampling strategy, the standard normal space with the radius beyond first order reliability index is divided into multiple spherical layers, which are then sampled by the reconstructed vector layer by layer. Combined with the full probability formula, a spherical layer sampling algorithm is developed to estimate the structural failure probability. Finally, three typical examples are taken as objects of interest, and the performance of the algorithm is verified through comparative analysis. The results show that, the proposed algorithm has high sampling efficiency and convergence performance, and the error of calculation results is within 3%. Compared with other algorithms, its estimation variance is smaller, and it can effectively solve complex reliability problems such as multiple design check points. The algorithm has advantages in sampling efficiency, scope of application, and stability, and is more suitable for solving and analyzing the reliability of actual complex structures.
-
地震动谱反演是频域上同时分离地震动震源、传播路径和场地效应的高效方法，该方法普遍应用于地震震源特征[1-4]、地壳介质衰减特性[5-6]、场地效应[7-8]、地震动不确定性[9]等的研究，这些研究对深入理解地震震源物理、探究地震发震机理、可靠预测地震动及地震危险性有着重要贡献.
根据传播路径衰减表示方式的差异，谱反演方法可分为参数化和非参数化两种. Castro等[10]首次提出的非参数化方法将传播路径衰减约束为距离的单调递减平滑曲线，既体现了传播路径衰减的复杂性，又描述了地震波在传播中的不断衰减，解决了参数化方法利用经验衰减模型会导致品质因子反演结果为极大值甚至负值，违背地震波衰减损耗的物理事实. 之后非参数化方法开始广泛应用于地震活跃地区的地震波衰减特征及地震震源特征等的研究，例如日本[2]、新西兰[11]、欧洲[3,9,12]、中国四川地区[13]等，相比参数化方法，近年来非参数化方法的应用更普遍.
尽管非参数化谱反演的应用十分普遍，但反演结果的可靠性需要谨慎处理诸多关键环节来确保. 谱反演必须考虑震源与场地项之间的权衡，一般通过选择合适的参考场地来实现，参考场地的选取显然会影响反演结果的可靠性，目前尚无研究针对如何选择合理的参考场地给出合理建议. 根据反演步骤的差异，非参数化谱反演具体分为两步法[10]和单步法[2]，两种方法的反演结果是否存在明显差异并不清楚，也未有研究评价应优先选用哪种方法. 根据理论震源模型[14]，震源谱以地震矩和拐角频率表示，因此震源谱的反演结果常用于地震震源参数的估计及震源物理特性研究，地震矩和拐角频率之间的权衡可能会影响震源参数估计的可靠性[15]，评估震源参数之间的权衡对其估计的影响十分必要.
针对影响地震动非参数化谱反演可靠性的关键环节，包括参考场地的确定、单步法或两步法的选择、震源参数估计，本文以2016—2017年意大利中部地震序列的地震动非参数化谱反演作为典型实例，欧洲强震动数据中心（engineering strong-motion，ESM）发布了地震序列八千余组人工处理的高质量的地震动记录，本研究限定记录峰值地面加速度（PGA）上限（100 cm/s2）、震源距上限（100 km）及台站（地震记录数下限）10 （10），选用了142个台站收集的78次震级（3.5～6.1）级地震的5135组观测记录用于谱反演研究，截取记录S波并在其前后增加余弦边瓣窗口消除截断误差，S波到达时刻和结束时刻分别通过人工识别和能量法[3]确定，最后以两水平方向S波傅里叶谱均方根代表观测记录水平向S波傅里叶谱. 本研究选用大量强震动记录可确保足够的数据冗余，避免因记录数过少造成反演结果的不可靠，通过系统分析反演结果，提出确保反演可靠性的上述关键环节的处理建议，可靠反演结果有助于地震震源物理的准确认识及地震动和地震危险性的有效预测.
1. 非参数化谱反演方法
第i个地震（震级Mi）中第j个台站（震源距Rij）观测到的地震动水平向S波的傅里叶幅值谱（Oij）可表示为
lnOij(f,Mi,Rij)=lnSi(f,Mi)+lnAij(f,Rij)+lnGj(f)， (1) 式中：f为频率；Si(f,Mi)为第i个地震的震源谱；Aij(f,Rij)为传播路径衰减；Gj(f)为第j个台站的场地效应.
两步法首先从S波傅里叶谱中分离传播路径衰减项（第1步），即
lnOij(f,Mi,Rij)=lnMai(f)+lnAij(f,Rij)， (2) 式中：Mai( f )为与第i次地震震级大小相关的量.
之后从传播路径衰减修正的S波傅里叶谱中同时分离震源和场地项（第2步），即
ln(Oij(f,Mi,Rij)/Aij(f,Rij))=lnSi(f,Mi)+lnGj(f). (3) 单步法直接求解式（1），从S波傅里叶谱中同时分离震源、传播路径衰减和场地项，求解时需约束传播路径衰减及震源和场地项的权衡.
2. 参考场地选择
参考场地一般为场地放大恒为1的无风化出露坚硬岩石场地[16]或平均场地放大为1的多个岩石场地[10]或至少一个放大效应已知的场地[17]. 实际应用中找到理想的出露坚硬岩石场地作为参考场地十分困难，多数研究采用场地放大效应恒为1的一个或多个岩石类场地作为参考场地[3,9,11].
一般考虑下列条件选取参考岩石场地：1） 场地地质资料，例如：场地类别、场地等效剪切波速（例如地下30 m土层的等效剪切波速Vs30）等；2） 水平/垂直（H/V）谱比曲线，岩石场地的谱比曲线在整个频段上平坦且幅值较小. 为保证反演结果的稳定性和可靠性，Bindi等[17]规定尽可能选取观测记录足够多且记录到震级和方位角范围足够大的岩石台站. Pacor等[3]则假定所有台站均为岩石场地且平均场地放大恒为1，利用谱反演给出所有台站的场地放大效应，再选取整个频段上场地放大小于2的场地作为参考岩石场地. Oth等[11]首先对参考岩石场地进行初选，通过震源谱和场地效应的谱反演试算结果从初选中确定. 鉴于理想岩石场地较难获取，上述这些研究提供了选取参考岩石场地的一系列方法，但这些方法均是不得已而为之，参考场地选取直接影响谱反演的可靠性.
根据Eurocode 8规范，本研究的142个观测台站中A类场地（岩石场地或其他接近岩石地质构造的场地，场地表面软弱层不大于5 m且Vs30 >800 m/s）台站共33个，其中7个为流动观测台站，A类场地台站作为参考场地的备选，因流动观测台站建设要求较低，受环境影响较大，7个流动观测台站不纳入备选之列. 26个备选台站中，分别取记录数≥ 50组、[40～50） 组、[30～40） 组、[20～30） 组、< 20组的台站分别为7、5、2、4和8个，有详细钻孔数据的3个（LSS、AQP和PSC，Vs30分别为1 091、836、1 000 m/s），只有LSS台站的观测记录充足（66组），AQP和PSC台站观测记录较少（分别为15组和14组）.
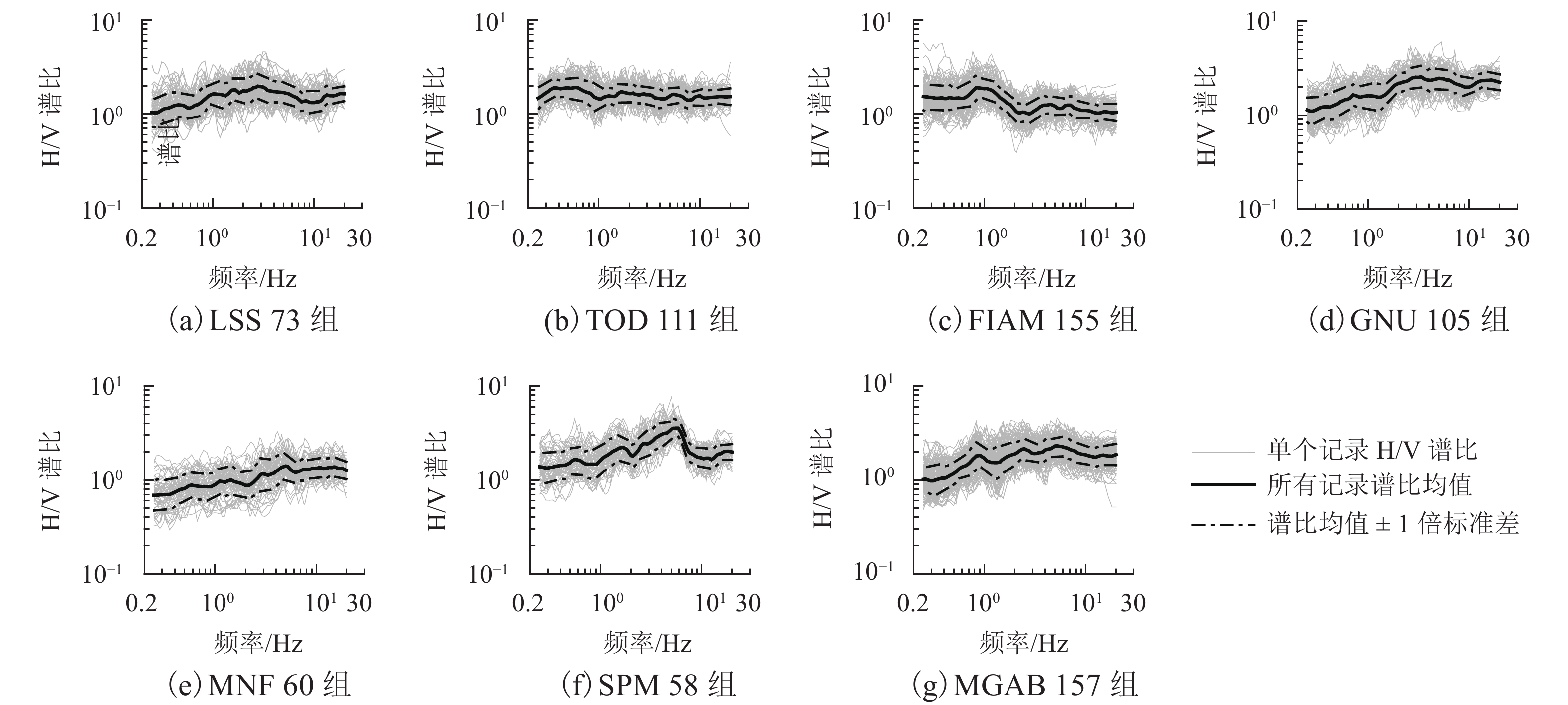
进一步缩小备选范围，仅以记录数不少于50组（73、111、155、105、60、58、157）的7个台站（LSS、TOD、FIAM、GNU、MNF、SPM和MGAB）作为备选，若最终证明均不合适，可适当放宽对备选台站记录数的要求. 分别利用7个台站的PGA≤ 50 cm/s2的观测记录计算0.25～20.00 Hz的H/V谱比曲线（见图1）. FIAM台站缺乏地形地貌描述且其H/V谱比曲线在1 Hz附近出现较为明显的峰值；SPM台站位于陡坡边缘，H/V谱比曲线幅值大且6.00 Hz附近出现明显峰值；GNU和MGAB台站的H/V谱比曲线幅值较大，说明这两个台站存在明显场地放大效应. 上述4个台站均不适合作为参考岩石场地. LSS台站的观测记录方位角范围广（大约100°）、记录震源距范围较大（>30 km）、H/V谱比曲线平坦且幅值小；TOD和MNF台站的H/V谱比曲线平坦且幅值小，此3个台站可作为初选的参考岩石场地.
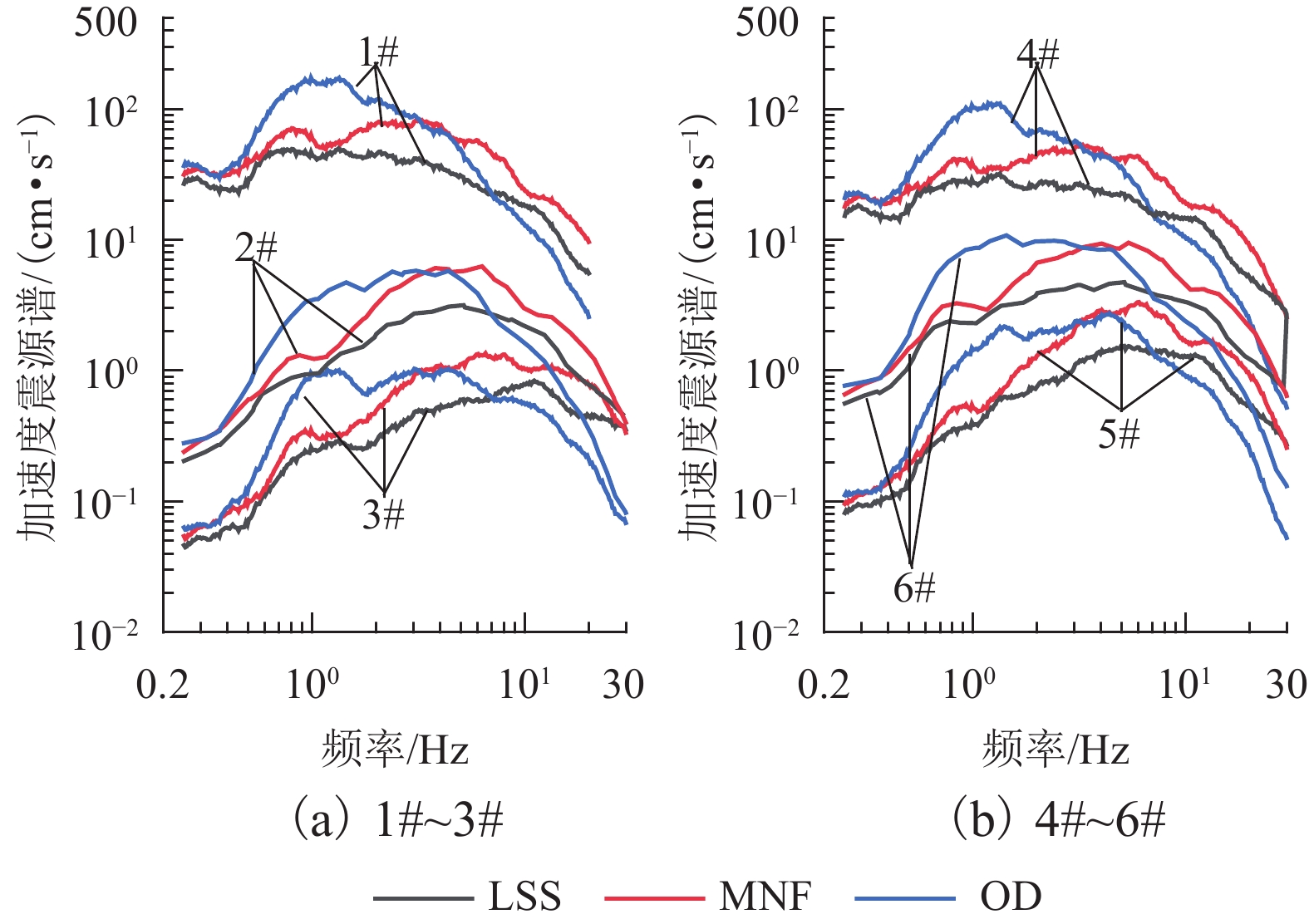
图 1 7个台站的H/V谱比曲线Figure 1. The horizontal-to-vertical spectral ratios at seven stations本研究以H/V谱比作为3个初选参考场地的场地放大效应，反演给出2016—2017年意大利中部地震序列观测记录的传播路径衰减、震源谱和场地效应，以LSS、TOD和MNF分别作为参考场地得到的6次典型地震的加速度震源谱如图2所示，6次地震（1#～6#）为20160824013632_ML6.0（2016年8月24日01：36：32发生的ML6.0级地震，余同）、20160825031716_ML4.5、20160824232205_ML3.8、20161026191806_ML5.9、20161031070545_ML4.1、20161103003501_ML4.8，震源谱大体上符合ω−2理论震源模型[14]，但不同参考场地得到的震源谱的形状及幅值差异明显. LSS和MNF台站作为参考场地的震源谱形状相似，LSS台站作为参考场地的震源谱的幅值更小；TOD台站作为参考场地的震源谱的形状明显不同，低频段（0.50～2.00 Hz）幅值更大，高频段（> 10.00 Hz）衰减更快. 上述结果清楚地展示了参考场地选取对反演结果的显著影响.
图 2 6个典型地震的加速度震源谱反演结果Figure 2. The inverted acceleration source spectra of six typical earthquakesS(f)=(2πf)2exp(−πfκ)CM0/[1+(f/fc)2], (4) 式中：C为常数，定义见文献[4]；M0为地震矩；fc为拐角频率.
网格搜索方法估计地震的震源参数（M0、fc）及高频衰减参数κ，根据M0计算矩震级Mw，利用M0、fc计算地震应力降Δσ[14].
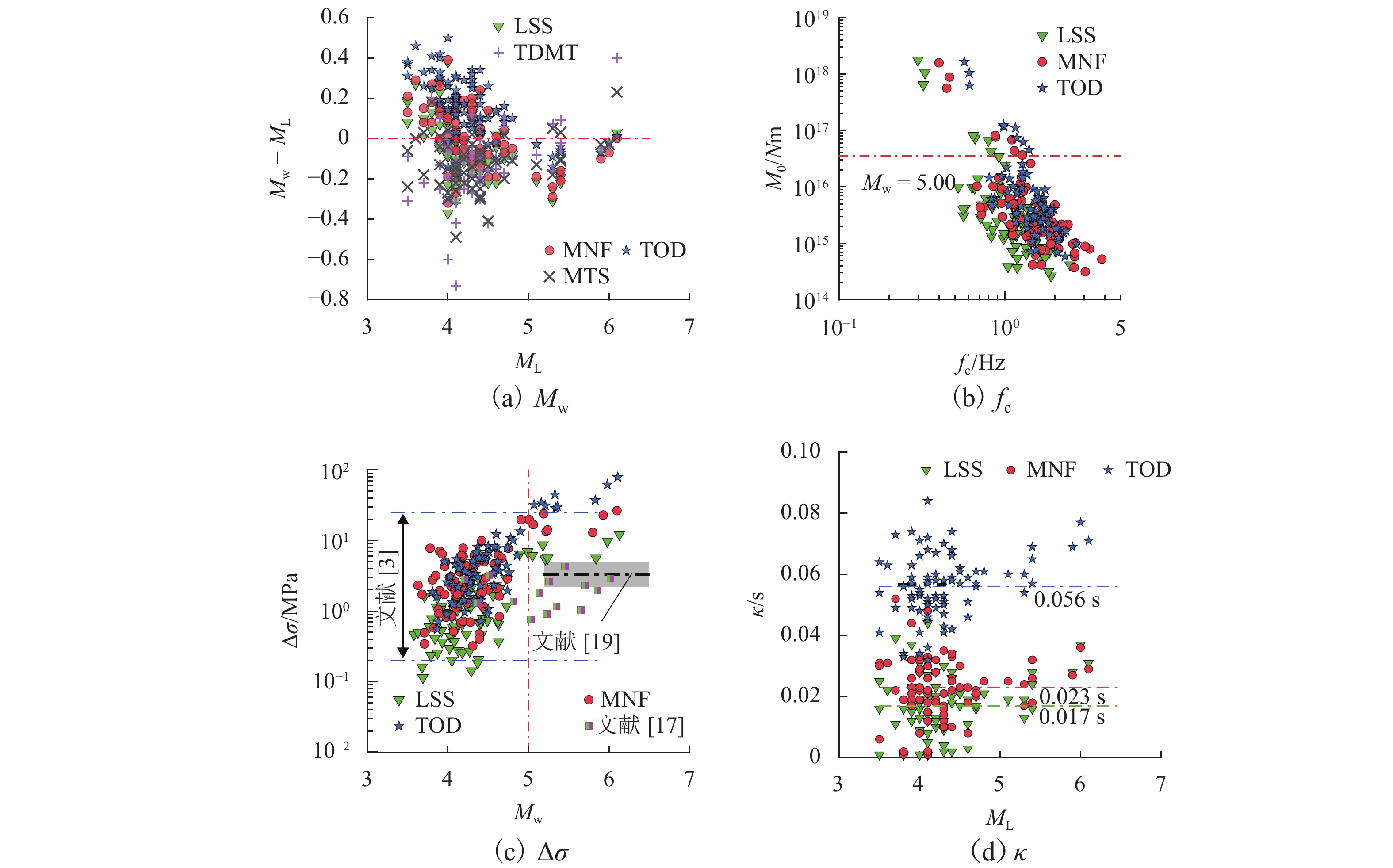
LSS、MNF和TOD台站分别作为参考场地时，利用反演震源谱得到的Mw估计值与INGV正式发布的地方震级ML的差值如图3（a）所示，整体上Mw估计值依次增大，LSS和MNF台站作为参考场地的Mw估计值接近，明显低于TOD台站作为参考场地的结果. ML< 5.3时，随震级增大，Mw估计值与ML之差整体上呈下降趋势，Mw估计值从明显高于ML逐渐变化为低于或接近ML；ML> 5.3时，Mw估计值普遍低于ML，且随ML增大，Mw与ML的差异逐渐缩小. 图3（a）同时比较了TDMT和MTS提供的Mw与ML之差. TDMT和MTS提供的Mw值普遍低于ML，LSS、MNF和TOD台站作为参考场地的Mw估计与TDMT、MTS提供的Mw存在一定偏差，尤其是小震的矩震级估计偏大，这可能与距离相关的能量法截取的小震记录S波窗口过大有关，但TOD作为参考场地的Mw估计与TDMT、MTS提供的Mw的偏差最显著.
图3（b）、（c）给出了LSS、MNF和TOD台站作为参考场地的fc和Δσ估计值，参考场地不同时fc和Δσ估计值差异明显，LSS作为参考场地的fc估计值明显低于MNF和TOD作为参考场地的结果，相应地LSS作为参考场地的Δσ估计值也最小. 参考场地不同时M0−fc和Δσ−Mw的变化趋势较为一致，Mw< 5.00时，Δσ估计值随Mw的增大而增大，LSS、MNF和TOD作为参考场地的Δσ估计值为0.11～2.90、0.32～9.97和0.55～13.45 MPa；Mw≥ 5.00时，Δσ估计值随Mw的变化不明显，LSS、MNF和TOD作为参考场地的Δσ为5.40～12.19、12.96～26.50和28.57～78.84 MPa，明显高于全球板间地震平均应力降（3.31 MPa）[19]及Bindi等[17]估计的1997—1998年Umbria-Marche地震序列（本研究选用地震序列的西北部）中15次Mw=4.20～6.00余震的应力降（图3（c））. Pacor等[3]利用非参数化谱反演确定的2009年L’Aquila地震序列（本研究地震序列的南部）应力降为0.25～25.00 MPa，与本研究选用LSS、MNF台站作为参考场地得到的Δσ估计值的范围较为一致（图3（c））.
LSS、MNF和TOD台站作为参考场地的κ估计值依次增大（图3（d）），κ估计值近似服从正态分布，LSS和MNF作为参考场地的κ估计值较为接近，均值分别为0.017 s和0.023 s，明显低于TOD作为参考场地的κ估计值（均值0.056 s）. κ估计值反映了参考场地中未予考虑的可能由于近地表岩层风化等引起的高频衰减[20]. 一般认为岩层风化越严重，其κ值越大. Oth等[2]利用日本井下钻孔记录反演得到的井下钻孔台站的κ均值为0.015 s，LSS和MNF台站作为参考场地的κ均值与日本井下钻孔台站κ均值接近，低于一般岩石场地的κ值（0.020～0.040 s），说明LSS和MNF台站近地表岩层风化不严重，而TOD作为参考场地的κ均值较高则说明该台站近地表岩层风化可能较为严重.
图 3 地震震源参数 （ Mw, fc and Δσ ） 及高频衰减参数 （ κ ） 估计值Figure 3. Estimated source parameters (Mw, fc and Δσ) and high-frequency decay parameters (κ)通过分析分别选用LSS、MNF和TOD台站作为参考场地的震源谱、震源参数及高频衰减参数的试算结果，LSS和MNF台站均可作为参考岩石场地的合理选择. 我们建议在选取参考场地时，首先综合考虑场地地质资料、观测记录H/V谱比曲线幅值和形状初步选择参考岩石场地，再分别以初选作为参考场地进行谱反演，根据震源谱、震源参数、高频衰减参数的试算结果，最终选取合适的参考场地.
3. 单步法与两步法
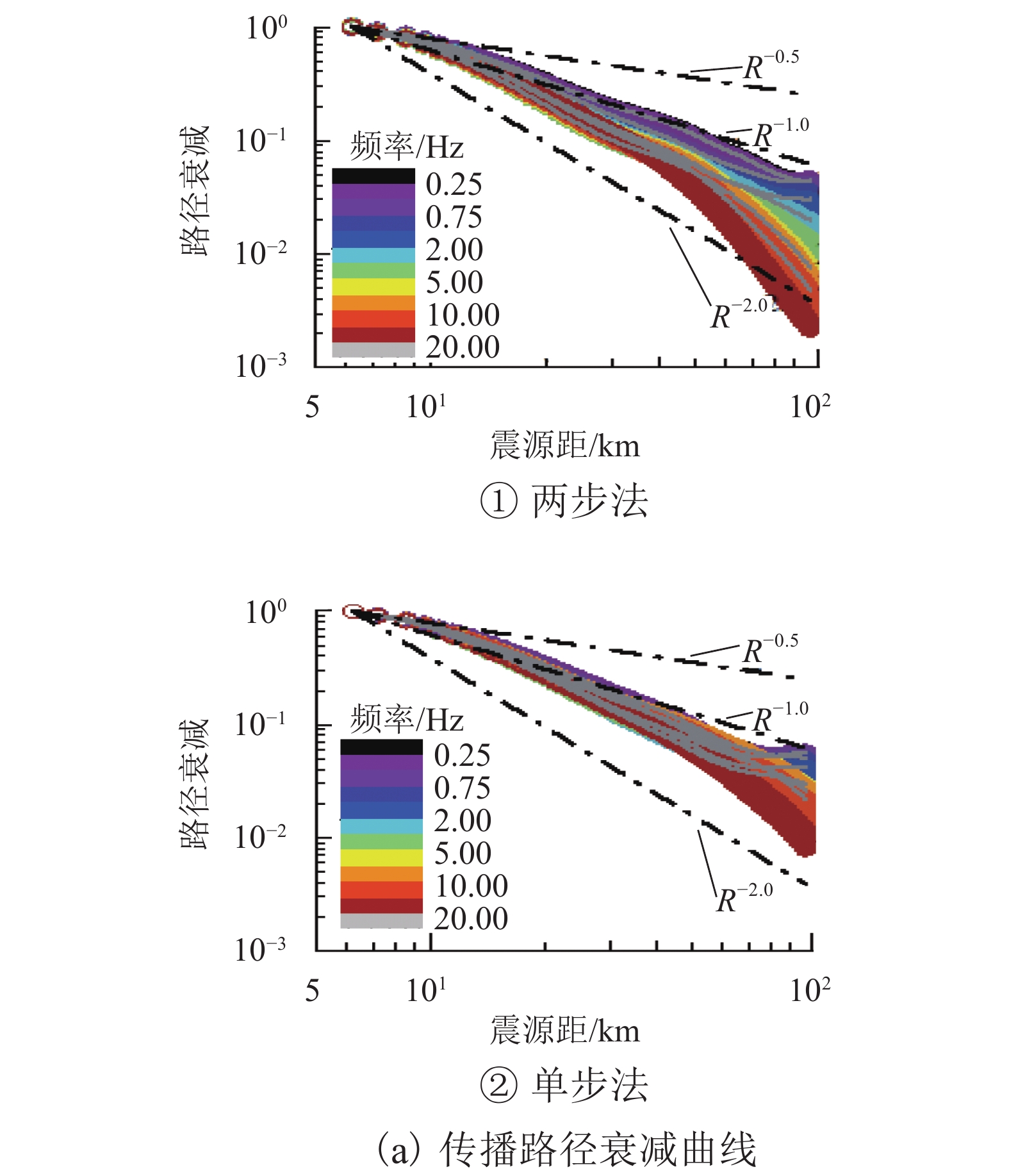
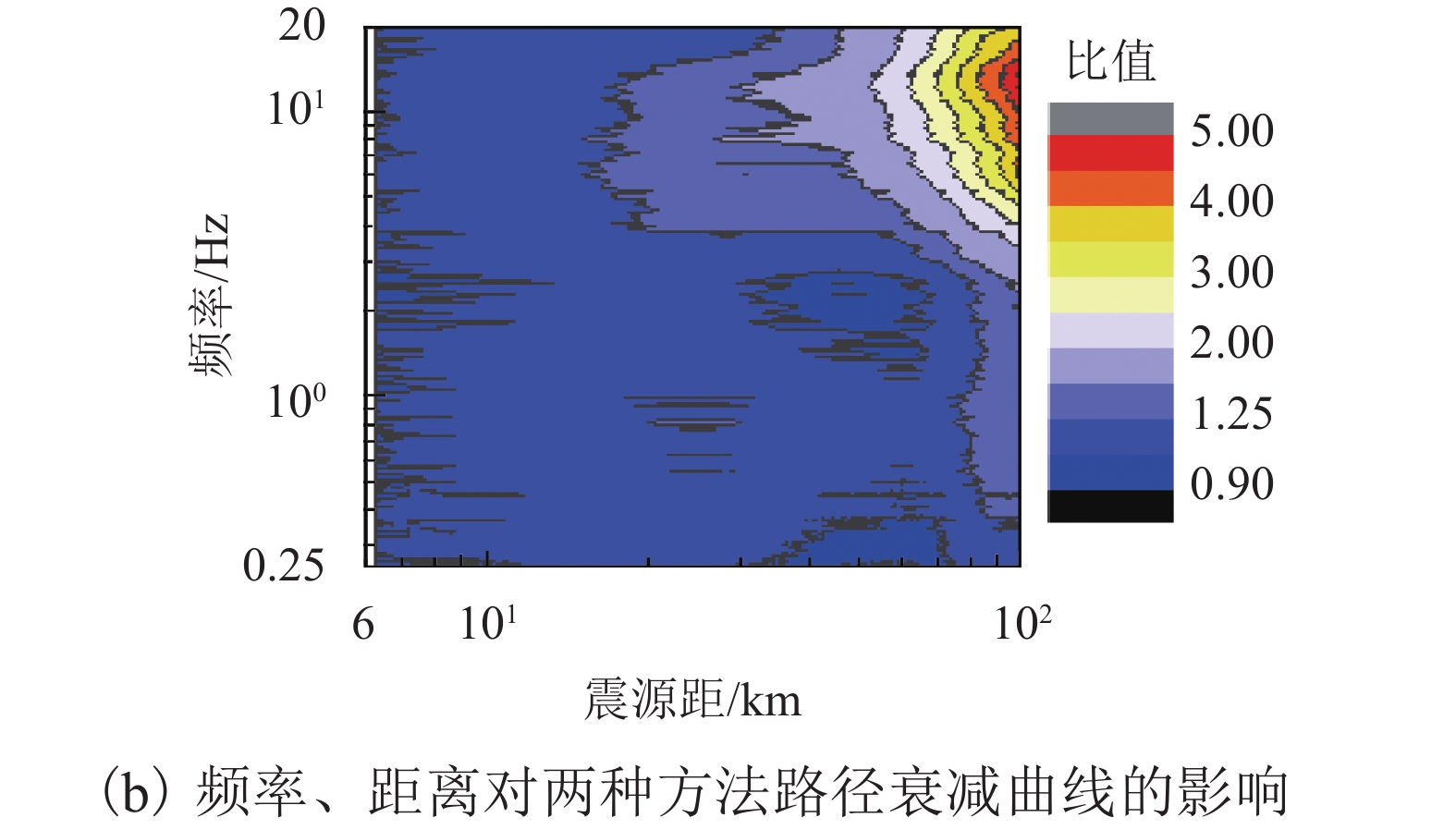
本研究分别采用两步法和单步法完成2016—2017年意大利中部地震序列地震动记录的非参数化谱反演，均以LSS台站作为参考场地. 两种方法分别确定的0.25～20.00 Hz传播路径衰减差异明显（图4（a）），差异大小与震源距、频率显著相关（图4（b）、（c））. 近场（< 20 km）或低频S波（< 2.00 Hz）的传播路径衰减较为一致，高频S波（> 5.00 Hz）传播路径衰减的差异随震源距增大迅速扩大，高频S波（> 5.00 Hz）在远场（> 60 km）的传播路径衰减的差异显著，两者相差可达5倍. 与单步法相比，两步法确定的传播路径衰减更强烈（数值更小）. 采用几何扩散和非弹性衰减简单表示传播路径衰减曲线，利用奇异值分解方法给出两种方法确定的传播路径衰减的几何扩散指数n和品质因子Q，两步法和单步法的n值较为一致，分别为 −1.01和 −1.07，品质因子的差异明显，分别为96.45f 0.59和212.45f 0.61，说明两种方法路径衰减差异主要为频率相关的非弹性衰减差异.
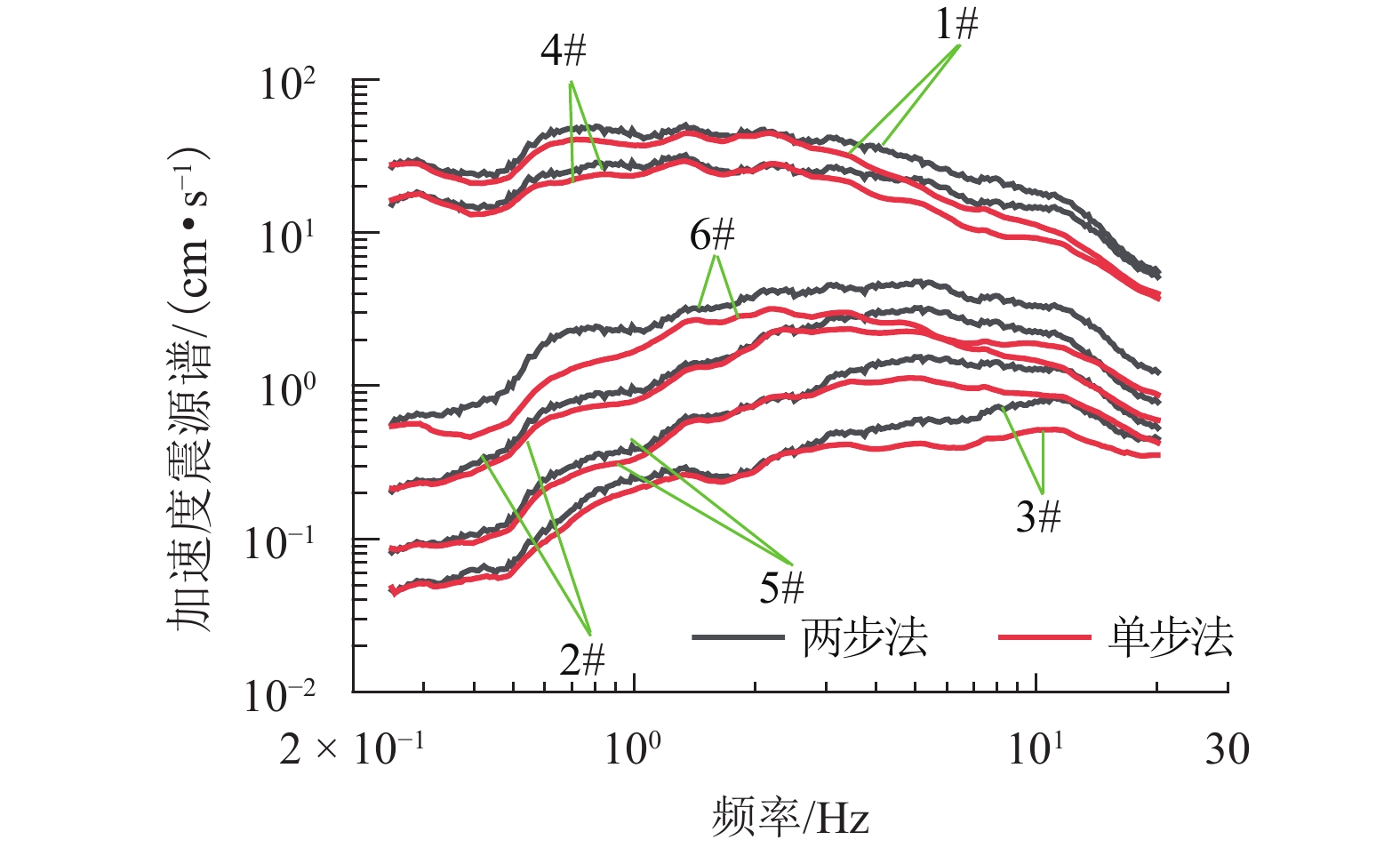
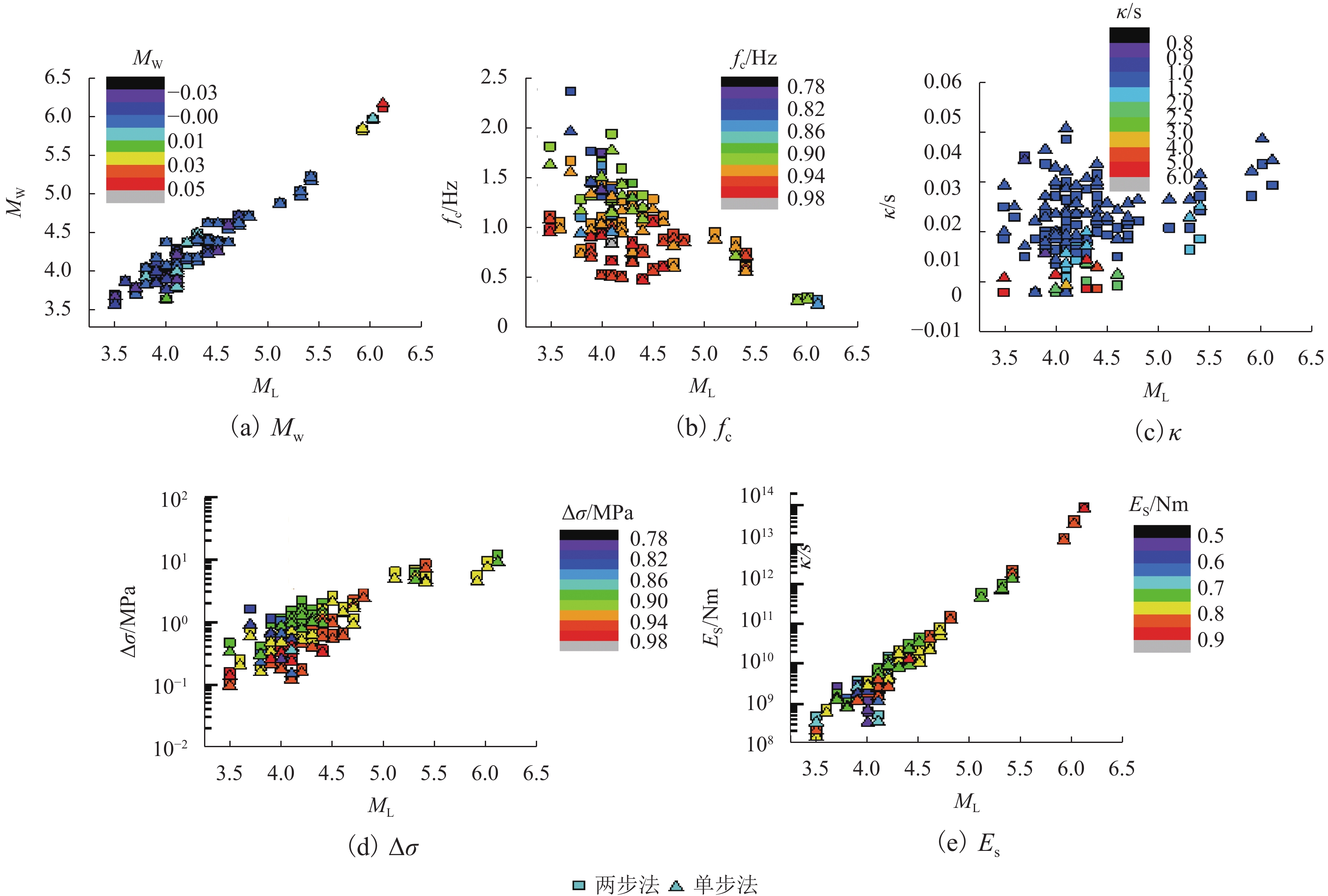
图 4 基于两步法和单步法反演路径衰减的差异Figure 4. Differences between path attenuations inverted by the two-step and one-step methods利用单步法和两步法分别确定的6次典型地震的加速度震源谱如图5所示，两步法的震源谱幅值普遍高于单步法，大于2.00 Hz的高频段尤为明显. 基于反演震源谱，利用网格搜索法估计地震震源参数（Mw、fc）及高频衰减参数（κ）（见图6（a）～（c）），两种方法Mw估计值相近，多数地震Mw估计值相差不超过0.02；两种方法fc估计值差异较小，单步法fc估计值整体上略小于两步法，多数地震单步法fc估计值约为两步法的88%～98 %，我们还注意到fc估计值的差异与fc大小有关，fc估计值的差异近似随fc增大而增大；两种方法κ估计值差异并不明显，单步法κ估计值普遍高于两步法，多数地震单步法κ估计值约为两步法的1.0倍～1.5倍，只有极少数κ估计值< 0.01 s的地震，单步法κ估计值约为两步法的2.0倍～6.0倍. 利用Mw和fc估计值计算得到Δσ（如图6（d））和辐射地震能量Es（如图6（e）），两种方法Δσ、Es估计值差异不明显，单步法Δσ和Es估计值均小于两步法，且大多数地震单步法Δσ和Es估计值约为两步法的70%～90%.
图 5 单步法和两步法反演给出的6次典型地震的加速度震源谱Figure 5. Acceleration source spectra for six typical events derived from the one-step and two-step methods
图 6 基于两步法和单步法的震源参数（Mw、fc、Δσ、Es）及高频衰减参数（κ）估计值的差异Figure 6. Differences in estimated source parameters (Mw, fc, Δσ and Es) and high-frequency decay parameter (κ) by the one-step and two-step methods非参数化谱反演的单步法和两步法给出的远场高频路径衰减差异显著，高频震源谱有一定差异，Mw、fc、Δσ、Es及κ的差异在可接受范围. 两步法将观测记录的震源和场地项假定为地震大小相关的量Ma，包含了该地震所有观测记录的平均震源谱和平均场地效应，单个观测记录的实际Ma与平均Ma存在偏差，每个距离分段的传播路径衰减会吸收该距离分段的所有观测记录的Ma偏差（主要来自场地项），如果该距离分段所有观测记录的Ma偏差均值明显偏离0，此时两步法传播路径衰减项出现偏差，推断这是两步法和单步法传播路径衰减偏差明显的根本原因. 由于高频路径衰减差异更显著，推断Ma在高频段的变化性更显著，可能与高频场地效应强变化性有关. 为避免两步法路径衰减被场地项干扰，我们建议尽可能采用单步法，但只研究地震震源特征时，由于震源参数影响较小，也可用两步法.
4. 震源参数求解中的权衡问题
常用网格搜索估计震源谱的特征参数（Mw、fc、κ），从一系列特征参数构建的理论震源谱中，利用网格搜索寻找最接近反演震源谱的一组特征参数表示的理论震源谱，作为震源谱特征参数的最佳估计. 估计Mw、fc和κ时，这些参数之间可能存在的权衡会影响结果估计的可靠性.
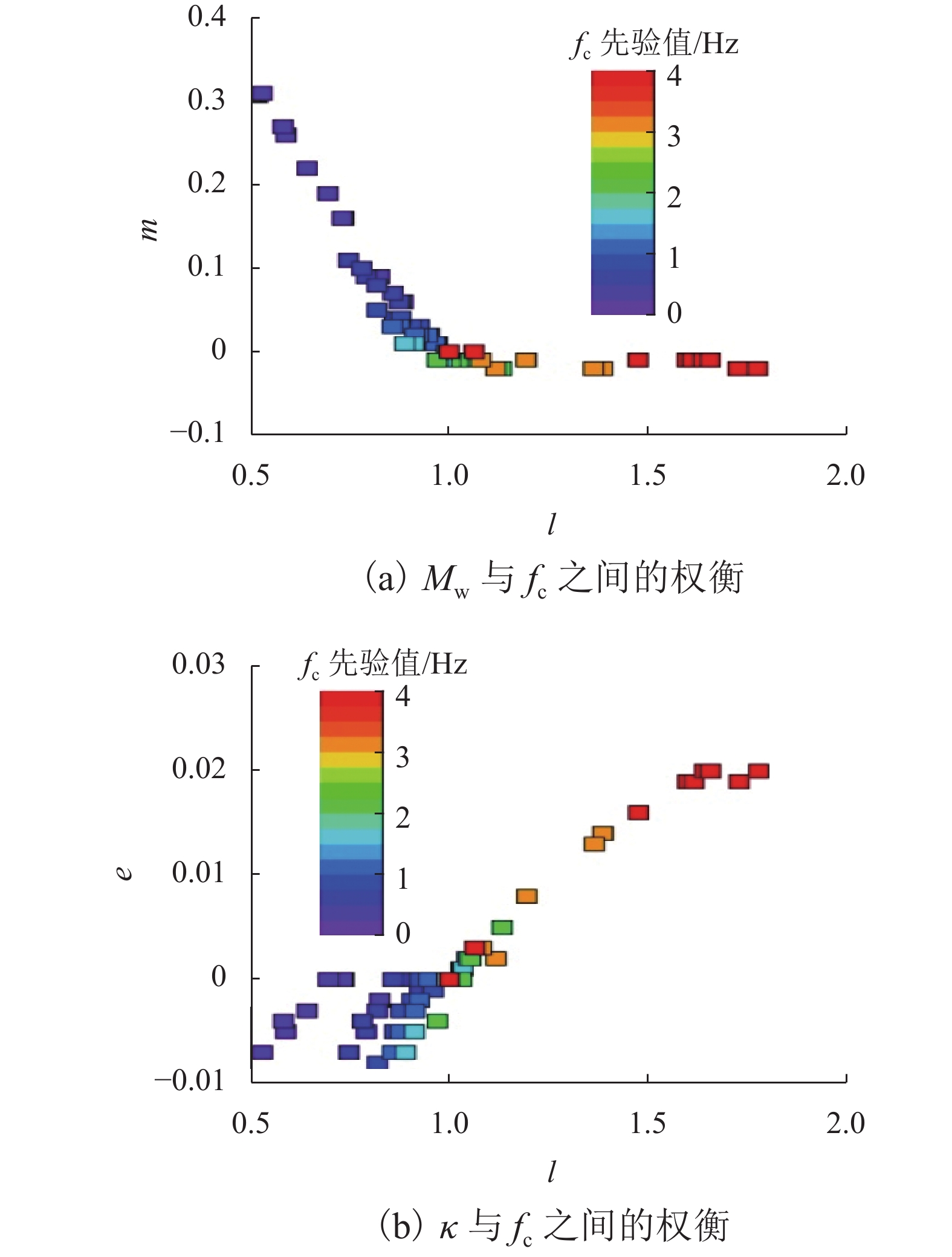
为评估这些参数之间的权衡，本研究利用一系列Mw、fc和κ先验值，根据式（4）、（5）构建光滑的先验震源加速度谱（352个），Mw取值3.75、4.25、5.00和6.00，fc取值0.3、0.5、0.8、1.0、1.5、2.0、3.0、4.0 Hz，κ取值为0～0.05 s范围内以0.005 s等间隔递增的一系列数值. 利用6次典型地震的理论震源谱与反演震源谱的残差修正先验震源谱，最终构建了2 112个先验震源谱. 网格搜索给出先验震源谱的Mw、fc和κ估计值，Mw估计值与先验值之差（m）、κ估计值与先验值之差（e）随fc估计值与先验值之比（l）的变化见图7.
fc先验值≤ 2.00 Hz时，fc估计值普遍偏小，而Mw估计值普遍偏大，fc估计值偏小的程度越显著则Mw估计值偏大的程度也越显著，这说明fc估计值越小则Mw估计值越大，两者之间存在一定的权衡. fc先验值为0.50～2.00 Hz 时，fc估计值约为先验值的80%～100%，Mw估计值与先验值相差小于 0.1，Mw、fc估计值与先验值的差异均较小，说明Mw和fc之间的权衡对Mw和fc估计值的影响有限. fc先验值大于 2.00 Hz时，fc估计值偏大而Mw估计值略有偏小，不论fc估计值偏大的程度如何Mw估计值偏小的程度近似不变，说明两者之间几乎不存在权衡. fc先验值≤ 2.00 Hz 时，fc和κ估计值均偏小，fc估计值越小κ估计值也越小；fc先验值 > 2.00 Hz，fc和κ估计值均偏大，fc估计值越大κ估计值也越大，说明fc与κ之间存在权衡. 由于κ主要控制震源谱的高频段，为避免fc与κ之间权衡的影响，确保Mw、fc的可靠估计，应尽量利用中低频段的震源谱估计Mw和fc.
图 7 震源谱特征参数之间的权衡Figure 7. Trade-offs among those parameters describing source spectra针对6次典型地震，κ为最佳估计值，Mw与fc的组合构建的理论震源谱与反演震源谱的拟合方差见图8. 图中：红色实线表示拟合方差最小值对应的fc值. 拟合方差的外包络线均呈开口向上抛物线，fc对应于最小拟合方差值，fc > 0.50 Hz 的4次地震，外包络抛物线在fc最佳估计附近谷值明显，容易识别；fc ≤ 0.50 Hz的2次地震，外包络抛物线在fc最佳估计值附近十分平坦，谷值不明显. 结果说明，fc > 0.50 Hz时，Mw和fc之间的权衡对Mw、fc估计影响很小，可不予考虑，fc ≤ 0.50 Hz时，需考虑Mw和fc之间的权衡，一般情况下fc较小地震的震级较大，其矩震级可直接采用震源机制解的结果以处理Mw和fc之间的权衡，确保fc的可靠估计.
5. 结 论
1） 反演结果与参考场地的选取密切相关，建议首先综合考虑台站观测记录数、场地地质资料、H/V谱比曲线等初选参考岩石场地，再以初选作为参考场地，根据震源谱、震源参数、高频衰减参数试算结果，最终选取合适的参考场地.
2） 单步法和两步法的远场高频路径衰减差异显著，高频震源谱有一定差异， Mw、fc、Δσ、Es及κ差异较小. 为避免两步法路径衰减被场地项干扰，建议采用单步法；如只研究地震震源特征，亦可采用两步法.
3） 网格搜索估计震源参数（Mw、fc）和高频衰减参数（κ）时，fc和κ之间存在权衡，为了尽可能避免此影响，建议利用中低频段的震源谱估计Mw和fc确保其可靠估计. fc < 2.00 Hz时Mw和fc之间存在一定权衡，但fc > 0.50 Hz时，此权衡对Mw、fc估计影响很小，fc较小时地震的震级较大，建议利用Mw处理Mw和fc之间的权衡，提高fc估计的可靠性.
致谢：中国地震局工程力学研究所基本科研业务费专项资助（2018B03）.
-
图 2 Zi抽样频率密度与标准正态分布概率密度对比
Figure 2. Fig. 2 Comparison of probability density between Zi sampling and standard normal distribution
图 3 n维标准正态空间中n−1维球面均匀分布
Figure 3. Spherical uniform distribution of n−1 dimensions in standard normal space
图 4 不同维度下Zi前四阶矩抽样结果
Figure 4. Sampling results of first four-order moments of Zi in different dimensions
图 8 算例1极限状态曲面及球层划分
Figure 8. Limit state surface and spherical layer division in standard normal space for case 1
图 9 算例1球层抽样失效概率变异系数随抽样次数变化
Figure 9. Coefficient of variation in failure probability of spherical layer sampling with sampling times for case 1
图 11 算例3钢斜拉桥最大单悬臂施工工况示意（单位：m）
Figure 11. Illustration of case 3 steel cable-stayed bridge in longest single cantilever construction phase （unit: m）
表 1 算例1不同方法计算结果对比
Table 1. Comparison of calculation results with different sampling methods for case 1
计算方法 抽样
数/次Pf/×10−3 βform 相对误差/% 变异
系数HL-RF 1.832 2.9057 38.3 重要抽样 1000 2.090 2.8642 29.6 0.360 线抽样 1000 1.957 2.8850 34.1 0.004 球层抽样 1000 2.998 2.7480 0.9 0.062 MCS 1×106 2.970 2.7511 注：各算法变异系数结果为重复100次计算得到，后续算例同. 
下载: 导出CSV
表 2 算例2随机变量的分布参数
Table 2. Distribution parameters of random variables for case 2
项目 q/
（kN•m−1）ls/m As/m2 Ac/m2 Es/
GPaEc/
GPa均值 20 12 9.82 × 10-4 0.04 100 20 变异系数 0.07 0.01 0.06 0.12 0.06 0.06
下载: 导出CSV
表 3 算例2不同方法计算结果对比
Table 3. Comparison of calculation results with different sampling methods for case 2
下载: 导出CSV
表 4 算例3抗力不确定性变量分布参数
Table 4. Distribution parameters of resistance random variables for case 3
项目 km kf kp t≤16 mm 16 mm<t≤25 mm 25 mm<t≤38 mm 38 mm<t≤50 mm 偏差系数 1.1511 1.1228 1.0991 1.2074 1.0000 1.0890 变异系数 0.0656 0.0670 0.0843 0.0917 0.0200 0.1080
下载: 导出CSV
表 5 算例3荷载变量分布参数
Table 5. Distribution parameters of temporary load random variables during construction for case 3
项目 桥面吊机和焊接设备 焊接平台 张拉平台 均值/kN 2402 150 399 变异系数 0.0500 0.0500 0.0500
下载: 导出CSV
-
[1] 吕震宙,宋述芳,李洪双,等. 结构机构可靠性及可靠性灵敏度分析[M]. 北京: 科学出版社,2009: 13-85,194-242. [2] MELCHERS R E. Importance sampling in structural systems[J]. Structural Safety, 1989, 6(1): 3-10. doi: 10.1016/0167-4730(89)90003-9 [3] GEYER S, PAPAIOANNOU I, STRAUB D. Cross entropy-based importance sampling using Gaussian densities revisited[J]. Structural Safety, 2019, 76: 15-27. doi: 10.1016/j.strusafe.2018.07.001 [4] KOUTSOURELAKIS P S, PRADLWARTER H J, SCHUËLLER G I. Reliability of structures in high dimensions, part Ⅰ: algorithms and applications[J]. Probabilistic Engineering Mechanics, 2004, 19(4): 409-417. doi: 10.1016/j.probengmech.2004.05.001 [5] SCHUËLLER G I, PRADLWARTER H J, KOUTSOURELAKIS P S. A critical appraisal of reliability estimation procedures for high dimensions[J]. Probabilistic Engineering Mechanics, 2004, 19(4): 463-474. doi: 10.1016/j.probengmech.2004.05.004 [6] 吕召燕,吕震宙,张磊刚,等. 基于条件期望的改进线抽样方法及其应用[J]. 工程力学,2014,31(4): 34-39.LÜ Zhaoyan, LÜ Zhenzhou, ZHANG Leigang, et al. An improved line sampling method and its application based on conditional expectation[J]. Engineering Mechanics, 2014, 31(4): 34-39. [7] AU S K, BECK J L. Estimation of small failure probabilities in high dimensions by subset simulation[J]. Probabilistic Engineering Mechanics, 2001, 16(4): 263-277. doi: 10.1016/S0266-8920(01)00019-4 [8] AU S K, WANG Y. Engineering risk assessment with subset simulation[M]. Singapore: Wiley, 2014: 157-204. [9] KATAFYGIOTIS L S, CHEUNG S H. Application of spherical subset simulation method and auxiliary domain method on a benchmark reliability study[J]. Structural Safety, 2007, 29(3): 194-207. doi: 10.1016/j.strusafe.2006.07.003 [10] KATAFYGIOTIS L, CHEUNG S H, YUEN K V. Spherical subset simulation (S³) for solving non-linear dynamical reliability problems[J]. International Journal of Reliability and Safety, 2010, 4(2/3): 122-138. doi: 10.1504/IJRS.2010.032442 [11] NOWAK A S, COLLINS K R. Reliability of structures [M]. 2nd edition. New York: CRC Press, 2013: 97-100,126-161. [12] 何平. 数理统计与多元统计[M]. 成都: 西南交通大学出版社,2004: 18-27. [13] 盛骤,谢式千,潘承毅. 概率论与数理统计[M]. 4版. 北京: 高等教育出版社,2008: 106-110,135-208. [14] 巩祥瑞,吕震宙,孙天宇,等. 一种新的矩独立重要性测度分析方法及高效算法[J]. 北京航空航天大学学报,2019,45(2): 283-290.GONG Xiangrui, LÜ Zhenzhou, SUN Tianyu, et al. A new moment-independent importance measure analysis method and its efficient algorithm[J]. Journal of Beijing University of Aeronautics and Astronautics, 2019, 45(2): 283-290. [15] GONG W P, JUANG C H, MARTIN J R II, et al. New sampling method and procedures for estimating failure probability[J]. Journal of Engineering Mechanics, 2016, 142(4): 04015107.1-04015107.10. [16] DER KIUREGHIAN A, DAKESSIAN T. Multiple design points in first and second-order reliability[J]. Structural Safety, 1998, 20(1): 37-49. doi: 10.1016/S0167-4730(97)00026-X [17] 白冰. 大跨度钢斜拉桥施工及运营过程系统可靠度研究[D]. 成都: 西南交通大学,2015. [18] CREMONA C. Structural performance: probability-based assessement[M]. London: ISTE,2011. [19] 薛国峰. 结构可靠性和概率失效分析数值模拟方法[D]. 哈尔滨: 哈尔滨工业大学,2010. 期刊类型引用(2)
1. 吴迪，李艾文，李丹，贾龙，韦学英，吴建建. 岩溶路基加筋防塌治理的简化设计方法. 中国岩溶. 2023(03): 538-547 . 
百度学术2. 陈玲玲，马露，王志远. 动荷载下桩承式路堤中加筋体的作用机理. 宜春学院学报. 2020(09): 53-56+102 . 百度学术其他类型引用(4)
-
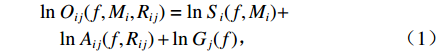
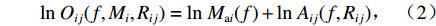
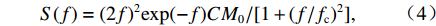
下载:
下载:
百度学术
点击查看大图
计量
- 文章访问数: 179
- HTML全文浏览量: 234
- PDF下载量: 18
- 被引次数: 6