Influence of Flexibility Characteristics of Levitation Chassis on Curve Negotiation Performance of High-Speed Maglev Vehicle
-
摘要:
为研究高速磁浮悬浮架小曲线通过动力学性能,考虑高速磁浮悬浮架柔性振动,建立悬浮架有限元模型,并计算其弹性模态,建立高速磁浮整车车辆动力学模型;应用同济大学磁浮试验线线路条件、试验速度曲线及拟合的轨道不平顺,分析了悬浮架柔性振动对悬浮、导向电磁铁间隙、电磁力的影响;同时,建立了刚性悬浮架动力学模型与之对比. 研究结果表明:R400小曲线通过时,电磁铁动力学性能受悬浮架柔性振动的影响较大,两种模型的导向力相差约12.5 kN,悬浮力相差约6.0 kN;通过试验仿真比较,考虑悬浮架柔性的计算结果更接近于实测结果;悬浮架垂向和横向振动的主频分别为10.4 Hz和13.2 Hz,分别与前后悬浮框相对点头、反相摇头模态频率相近;在研究控制参数优化、悬挂参数优化、运行稳定性等高速磁浮关键问题时应考虑悬浮架的柔性振动.
Abstract:In order to investigate the small curve negotiation performance of high-speed maglev trains, the flexible vibration of the levitation chassis is explored, and the finite element model of levitation chassis is established to calculate its elastic modes; then the dynamics model of the high-speed maglev vehicle is built. According to the track conditions, speed curve and fitted track irregularities from Tongji University’s maglev test line, the influence of flexible vibration of levitation chassis is analyzed on the gap and electromagnetic force of guidance and levitation electromagnet. Meanwhile, a dynamics model of a rigid levitation chassis is built for comparison purpose. The results show that the dynamic performance of electromagnet is greatly affected by flexible vibration of the levitation chassis when the negotiating curve has a smaller radius of 400 m. The difference of the guidance force between the two models is about 12.5 kN, while the difference of the levitation force is 6.0 kN or so. The comparison with the simulation demonstrates that the results from the model of the levitation chassis flexibility is more close to the test results. The main frequencies of the vertical and lateral levitation chassis vibration are 10.4 Hz and 13.2 Hz respectively, which are similar to modal frequencies of relative pitching and anti-phase yawing between the front and rear levitation frame. The flexibility of levitation chassis should be taken into account in the key issues of high-speed maglev trains, such as control parameter optimization, suspension parameter optimization, and running stability.
-
Key words:
- maglev vehicle /
- curve negotiation /
- levitation chassis /
- flexible vibration /
- electromagnet
-
时速600 km常导高速磁浮列车系统由车体和悬浮架两个子系统构成,其中悬浮架子系统是重要的承载部件,是安装电磁铁、二系悬挂系统等部件的基础,传递牵引力、悬浮力、导向力以及车体载荷. 为了解决在缓和线路轨道扭转所形成的多点约束问题,在结构上通过设置提供较小扭转刚度的纵梁,实现前后悬浮框相对扭转的机械解耦,以保证高速通过曲线的性能.
目前,常导磁浮车辆系统动力学研究主要围绕控制算法的研究和车轨耦合动力响应研究两个方向. 首先,研究学者期望设计一种动态性能优越的控制算法来提高控制系统的鲁棒性和自适应性,以提高磁浮列车主动控制的稳定性并降低车轨耦合振动[1-6];其次,结合多体动力学理论和控制理论,研究磁浮车辆系统以及车轨系统的动力响应[7-12]. 以上研究均将磁浮车辆动力学模型考虑为多刚体结构.
关于高速磁浮列车曲线通过时考虑悬浮架刚柔耦合动力学研究较少. 闫一凡等[13]基于UM建立的高速磁浮柔性悬架和刚柔耦合整车模型开展了振动传递仿真分析,并同多刚体整车模型对比分析,没有考虑控制系统和曲线通过的影响. 赵春发等[14-15]建立了包含车辆、控制系统、柔性悬浮架的TR08高速磁浮车辆刚柔耦合动力学模型,采用状态观测器的气隙-加速度反馈控制,计算了其动态响应,但是忽略了轨道几何不平顺激扰的影响. 以上文献中的电磁力模型均采用一维简化模型,忽略了磁阻、磁极数量及布置的影响.
建立考虑悬浮架柔性特征的高速磁浮整车车辆动力学模型,采用考虑磁极分布及铁芯、气隙磁阻的电磁力模型,应用同济大学磁浮试验线线路条件、试验速度曲线及拟合的轨道不平顺,分析了悬浮架柔性振动对悬浮、导向电磁铁间隙、电磁力的影响,并与刚性悬浮架进行对比分析. 同时,开展间隙的计算值与现车测试数据对比,并分析了悬浮架横向和垂向振动特征.
1. 高速磁浮车辆动力学模型
1.1 悬浮架
悬浮架由前后两个悬浮框、纵向连接结构组成,其中悬浮框主要包括横梁、摇臂、摇臂支梁、空气弹簧支梁、托臂等;纵向连接结构主要包括纵梁、牵引拉杆等,各部件由螺栓及铆钉连接,见图1.
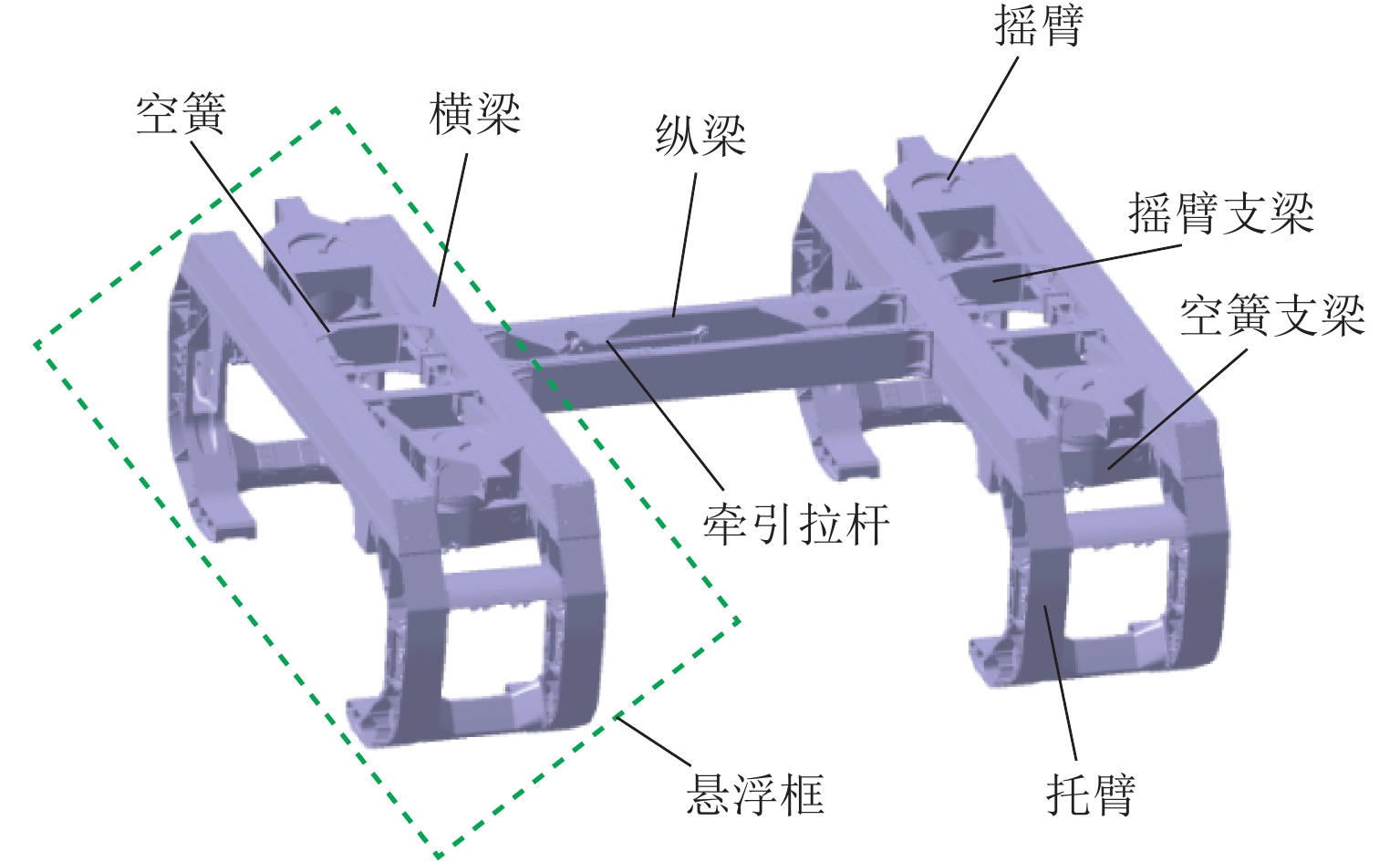
在刚性悬浮架模型中,为了模拟前后悬浮框相对扭转,将悬浮架分为前悬浮框、后悬浮框、纵梁3个体,在悬浮框和纵梁之间设置球铰,允许侧滚、点头、摇头3个方向的相对转动,并分别设置相应的扭转刚度,其中侧滚扭转刚度为1.05 × 103 kN·m/rad,点头扭转刚度为4.1 × 103 kN·m/rad,摇头扭转刚度为1.0 × 105 kN·m/rad. 刚性悬浮架模型的具体拓扑结构见图2,图中所示的0号铰、1号铰、2号铰、7号铰、10号铰、4号力元、5号力元、43号力元具体使用及设置方法可参考SIMPACK帮助文档.
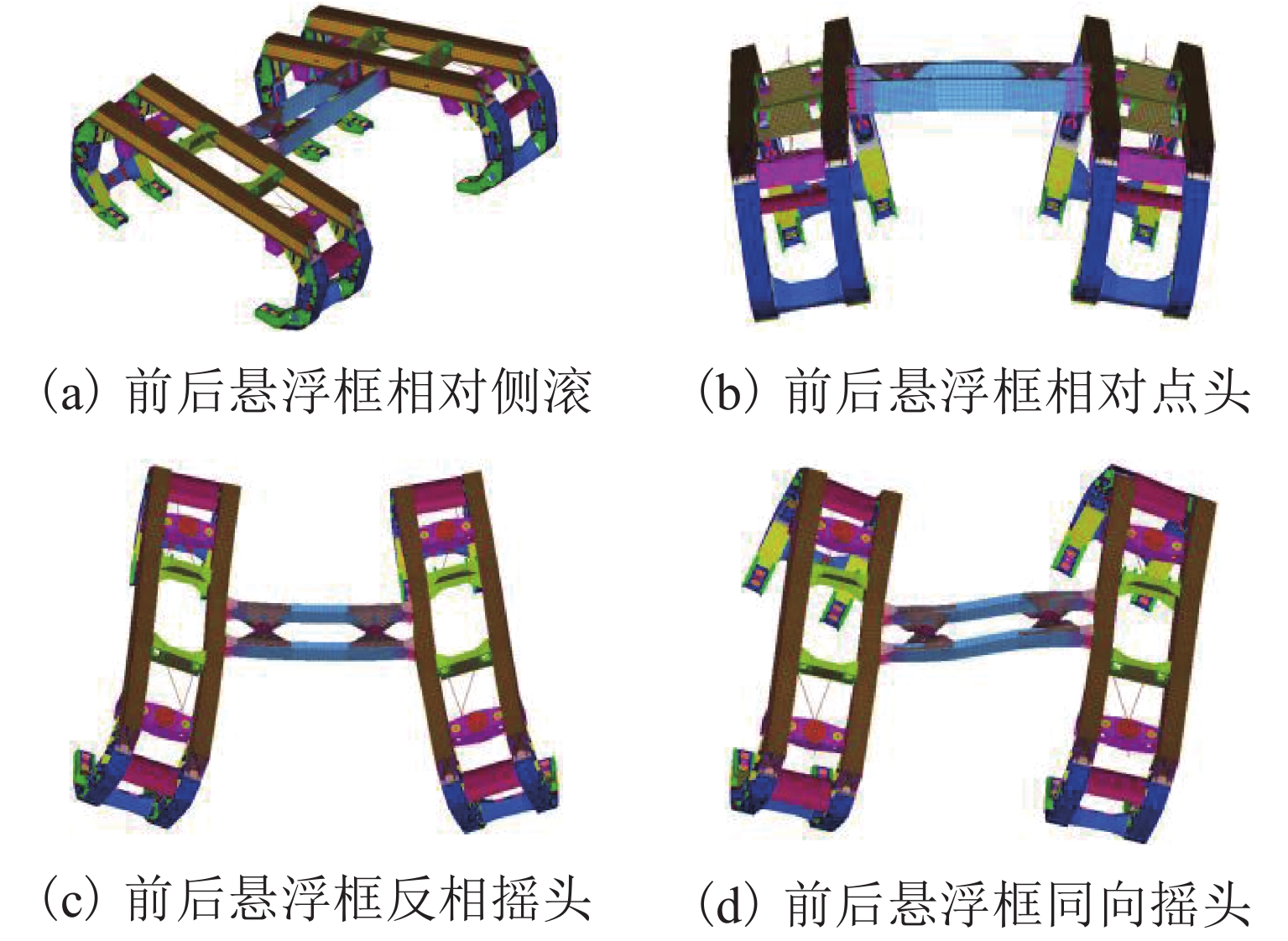
在柔性悬浮架模型中,首先建立除摇臂、摆杆之外的悬浮架有限元模型,设置其弹性模量为6.8 × 1010 Pa,泊松比0.3,密度2 660 kg/m3,进行自由模态分析,图3显示了前4阶模态振型. 然后将刚性悬浮架模型中的前后悬浮框和纵梁替换为以上所建的有限元模型,从而建立柔性悬浮架模型.
1.2 电磁铁
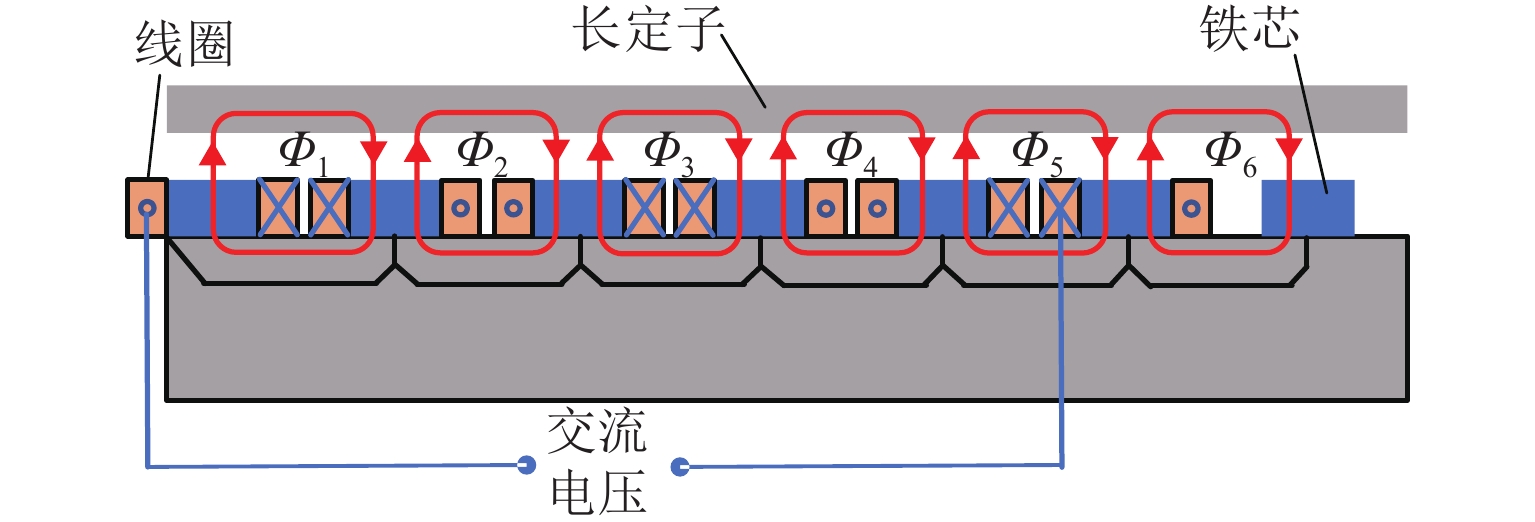
在结构设计方面,导向电磁铁和悬浮电磁铁很相似,因此以半个悬浮电磁铁(6磁极)为例,考虑磁阻和磁通的因素,推导电磁力公式. 此6个磁极是串联的,保证磁通以高的导磁率通过铁芯,见图4.
利用Maxwell准静态电场和磁场方程建立磁通计算的微分方程,由此得到[16]:
{{\boldsymbol{R}}_{{\text{mag}}}}{\boldsymbol{\varPhi }} = {\boldsymbol{\theta }}, (1) 式中:
{{\boldsymbol{R}}_{{\text{mag}}}} 为磁阻,{{\boldsymbol{R}}_{{\text{mag}}}} \in {\bf{R}^{6 \times 6}} ;{\boldsymbol{\varPhi }} 为磁通,{\boldsymbol{\varPhi }} = {\left[ {\begin{array}{*{20}{c}}{{\phi _1}} & {{\phi _2}} & \cdots & {{\phi _6}}\end{array}} \right]^{\rm{T}}} ;{\boldsymbol{\theta }} 为电磁电压,{\boldsymbol\theta} = {\left[ {\begin{array}{*{20}{c}}{{\theta _1}} & {{\theta _2}} & \cdots & {{\theta _6}}\end{array}} \right]^{\rm{T}}} ,对于每磁极来说,{\theta _k} = {n_k}I ,k = 1, 2,\cdots ,6 ,其中{n_k} 为线圈匝数,I为通过线圈的电流.利用Kirchhoff定律计算得到电压为
{U_{\text{M}}} = \sum\limits_{k = 1}^6 {\left( {{U_{{\text{ind}},k}} + {R_k}I} \right)}, (2) 式中:
{R_k} 为每个磁极线圈的电阻;{U_{{\text{ind}},k}} 为感应电压,并通过{U_{{\text{ind}},k}} = n{\dot \phi _k} 将式(1)和(2)关联.通过数值积分计算各个磁极的磁通,进而得到电磁力与磁通的关系式:[17]
{F_{{\text{mag}}}} = \sum\limits_{k = 1}^6 {{K_{{\text{mag}}}}{\phi _k}^2} , (3) 式中:
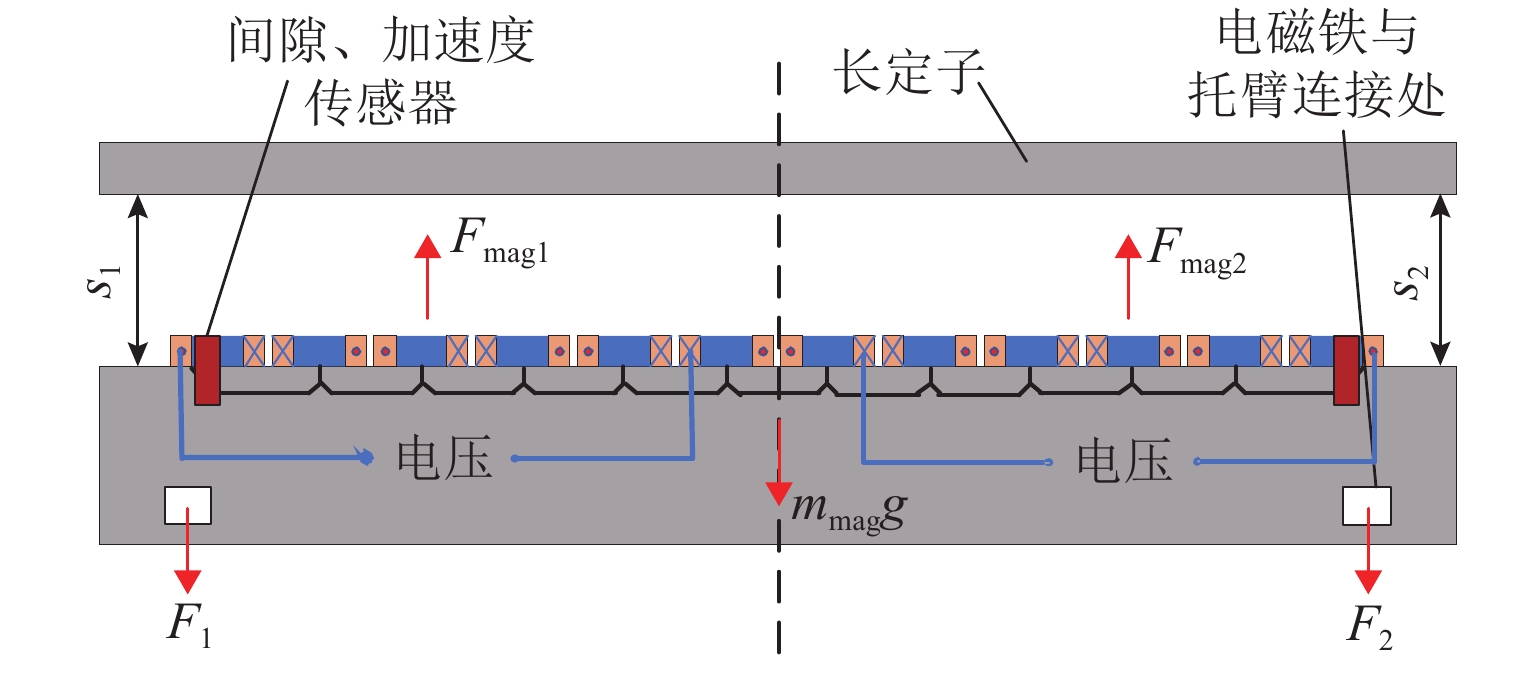
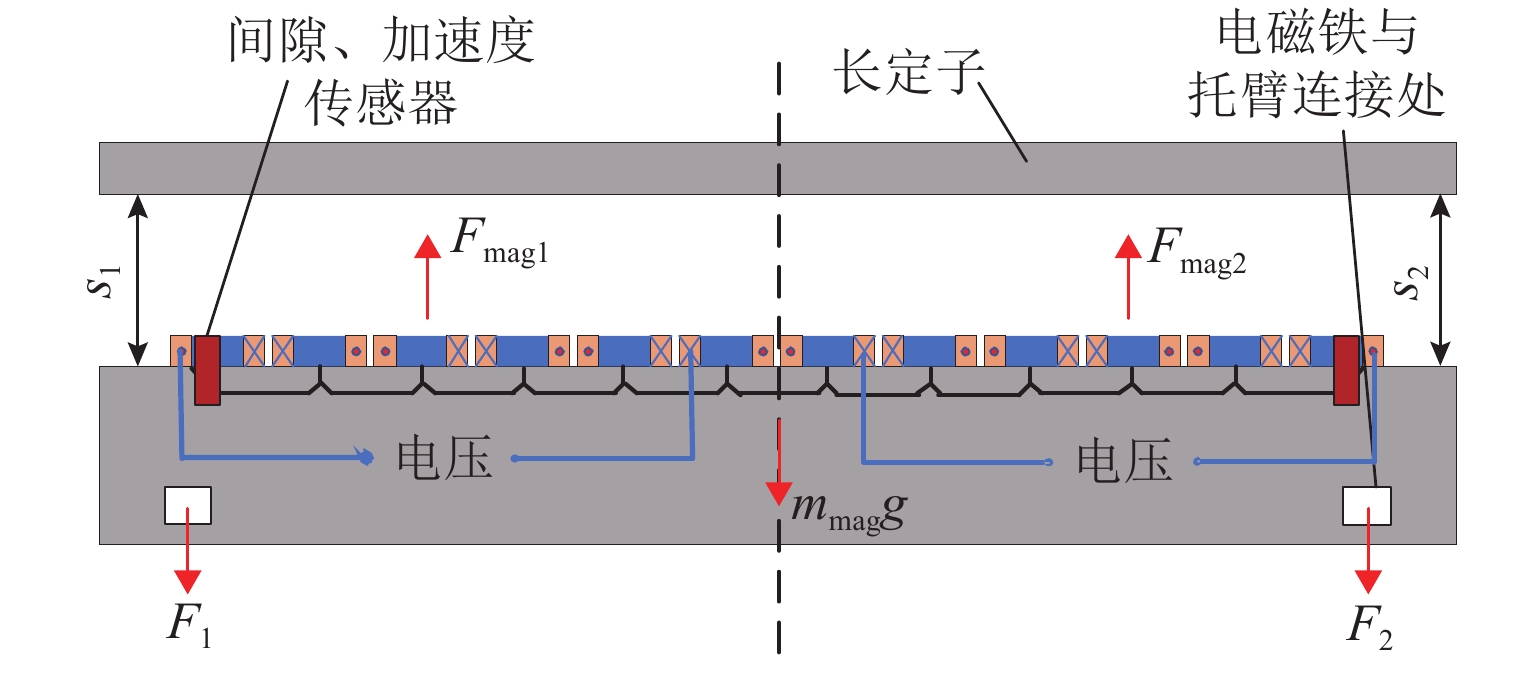
{K_{{\text{mag}}}} = \dfrac{1}{{2A}}\left( {\dfrac{1}{{{\mu _0}}} - \dfrac{1}{{{\mu _0}{\mu _{\text{r}}}}}} \right) ,{\mu _0} 为真空磁导率,{\mu _{\text{r}}} 为相对磁导率,A为铁芯对应的横截面积.{K_{{\text{mag}}}} 及所有磁阻的计算均需考虑电磁铁和长定子的材料属性及其精确的几何尺寸. 为了提高与车辆动力学模型联合仿真计算速度,通过参数拟合得到电压和电流之间的输入输出关系,将电磁铁的精细化模型等效为一个磁极的集中力模型,见图5,根据悬浮电磁铁受力分析,通过悬浮电磁铁的运动方程建立电磁铁磁路、电路、运动三者的耦合关系,如式(4).{m_{{\text{mag}}}}a = {m_{{\text{mag}}}}g + {F_1} + {F_2} - {F_{{\text{mag1}}}} - {F_{{\text{mag2}}}}, (4) 图5和式(4)中:
{m_{{\text{mag}}}} 为悬浮电磁铁的质量;a 为悬浮电磁铁的加速度;{F_j}\;\left( {j = 1,2} \right) 为悬浮架托臂对悬浮电磁铁的作用力;{F_{{\text{mag}}j}} 为一半悬浮电磁铁产生的电磁集中力;{s_j} 为一半悬浮电磁铁间隙测量值.1.3 整车动力学模型
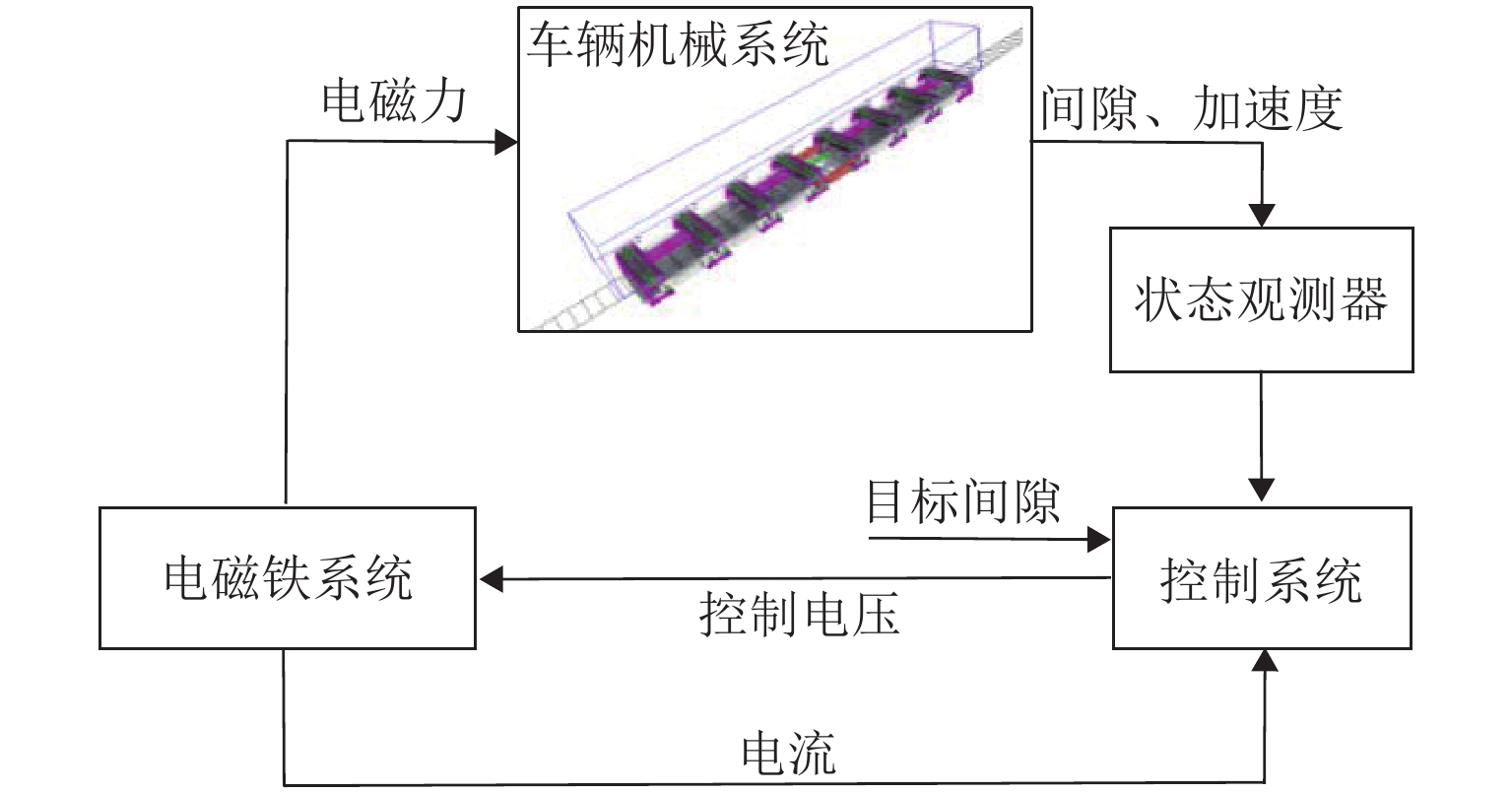
高速悬浮控制系统根据实测间隙及加速度的状态调节电磁铁的输入电压来实现电磁铁间隙跟踪目标间隙,以悬浮电磁铁控制策略为例说明具体实现方法[18],导向电磁铁的控制原理与之类似:悬浮电磁铁间隙传感器及加速度传感器得到的悬浮间隙和电磁铁加速度,经状态观测器处理后得到悬浮间隙、电磁铁速度、电磁铁加速度;根据悬浮间隙的目标值,计算实际偏差;与电磁铁电流一同进入控制系统,根据不同状态量的贡献度大小,设置相应的加权系数,综合计算得到电磁铁的控制电压,施加在电磁铁电路两端. 故考虑悬浮架柔性特征的高速磁浮整车车辆动力学模型架构见图6.
所用高速磁浮整车动力学主要参数见表1.
表 1 高速磁浮整车动力学主要参数Table 1. Main parameters in dynamics of high-speed maglev vehicles部件 物理量 数值 悬浮电磁铁 质量/kg 600 侧滚转动惯量/(kg·m2) 1.5 点头转动惯量/(kg·m2) 479.8 摇头转动惯量/(kg·m2) 480.7 导向电磁铁 质量/kg 390 侧滚转动惯量/(kg·m2) 3.1 点头转动惯量/(kg·m2) 0.3 × 103 摇头转动惯量/(kg·m2) 0.3 × 103 悬浮架 质量/kg 1.7 × 103 侧滚转动惯量/(kg·m2) 3.5 × 103 点头转动惯量/(kg·m2) 4.4 × 103 摇头转动惯量/(kg·m2) 7.4 × 103 车体 质量/kg 3.0 × 104 侧滚转动惯量/(kg·m2) 5.7 × 104 点头转动惯量/(kg·m2) 1.6 × 106 摇头转动惯量/(kg·m2) 1.6 × 106 悬浮电磁铁安装刚度 纵向/(N·m−1) 1.0 × 107 横向/(N·m−1) 4.8 × 105 垂向/(N·m−1) 1.2 × 107 侧滚刚度/(N·m·rad−1) 1.0 × 107 点头刚度/(N·m·rad−1) 1.0 × 107 摇头刚度/(N·m·rad−1) 1.6 × 103 导向电磁铁安装刚度 纵向/(N·m−1) 4.3 × 107 横向/(N·m−1) 2.5 × 105 垂向/(N·m−1) 4.4 × 107 侧滚刚度/(N·m·rad−1) 1.0 × 106 点头刚度/(N·m·rad−1) 1.0 × 106 摇头刚度/(N·m·rad−1) 1.0 × 106 空气弹簧 垂向刚度/(N·m−1) 2.0 × 105 横向刚度/(N·m−1) 1.1 × 105 辅助弹簧 横向刚度/(N·m−1) 3.0 × 105 限位弹簧 横向刚度/(N·m−1) 5.0 × 105 摆杆 垂向刚度/(N·m−1) 2.0 × 105 侧滚刚度/(N·m·rad−1) 1.0 × 104 2. 边界条件设置
2.1 线路条件
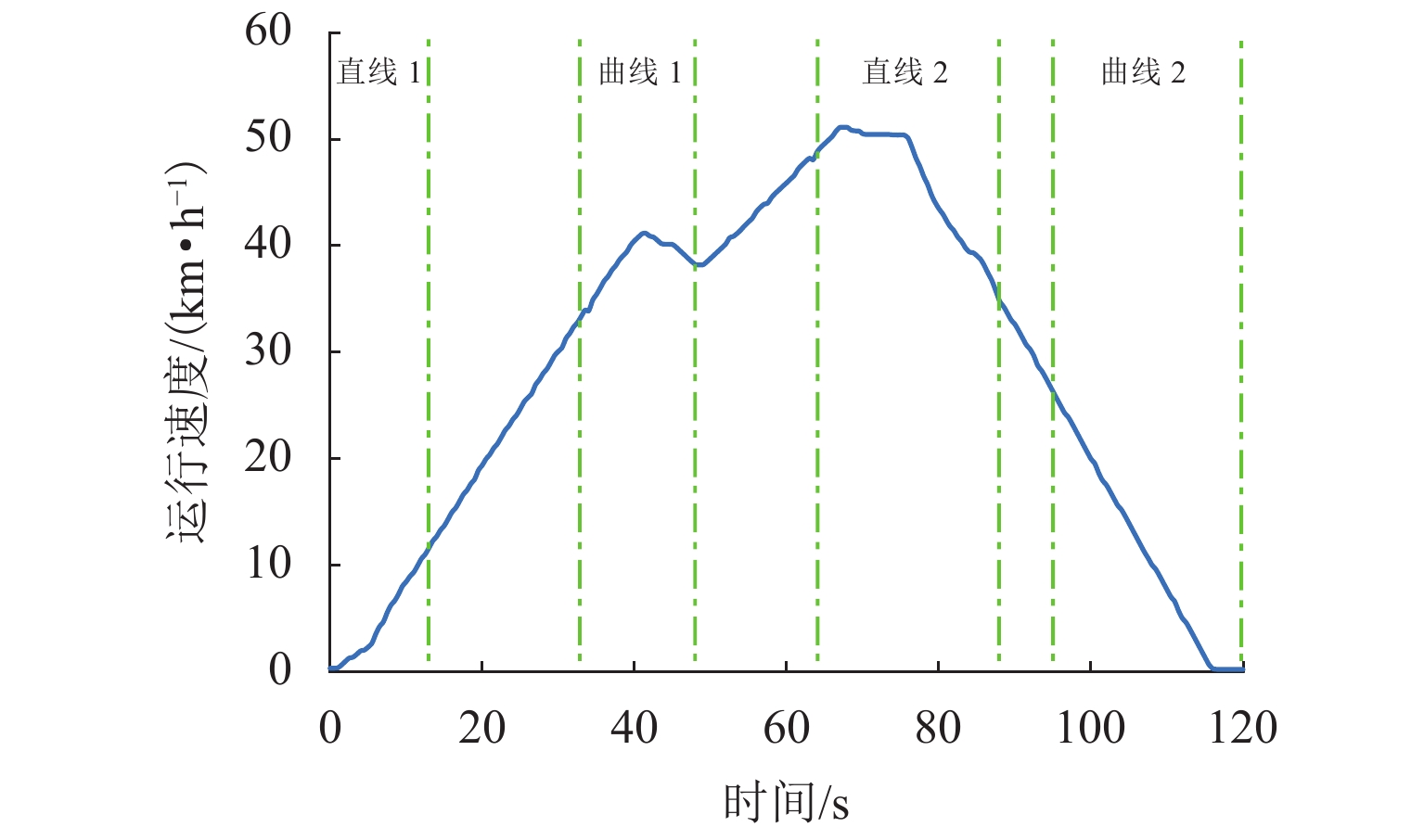
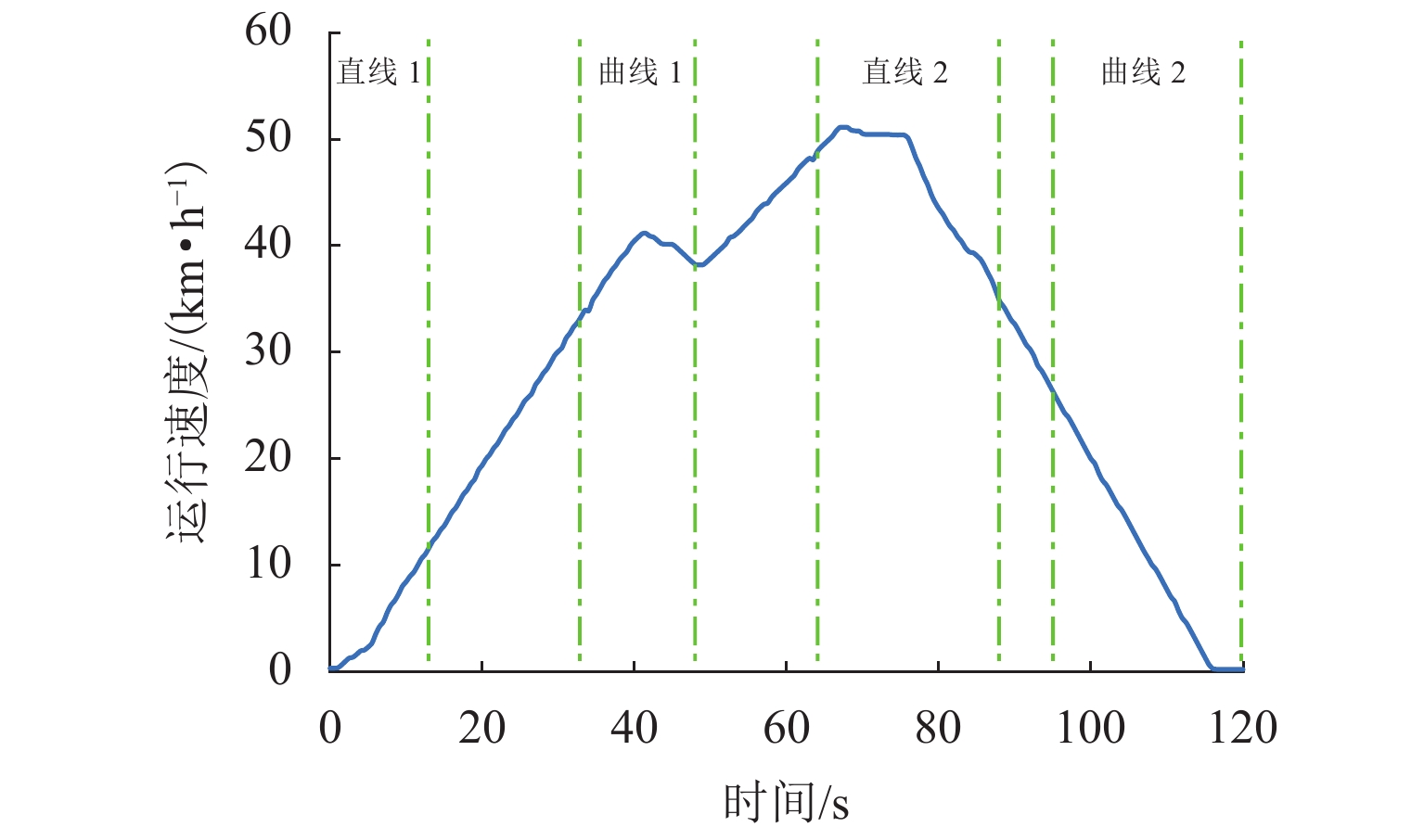
2020年6月由中车青岛四方机车车辆股份有限公司研制的时速600 km高速磁浮原理样车在同济大学试验线开展低速运行试验[19],其线路主要参数见表2. 根据试验线的线路条件及车辆实际运行速度曲线,分别设置了仿真计算所需要轨道线路信息及速度曲线,见图7.
表 2 同济大学高速磁浮试验线主要参数表Table 2. Main parameters about Tongji University’s maglev test line区间 长度/m 横坡角/(°) 曲线半径/m 坡度/‰ 直线 1 234 0 ∞ 0 曲线 1 443 3 400 0 曲线 2 340 2 1300 0 直线 2 550 0 ∞ 11.2 2.2 轨道不平顺
高速磁浮轨道不平顺除考虑部件安装公差外,结合实际工作中各功能面的安装组成引入其特征基础长度形成不平顺相关频率成分,同时考虑磁浮桥梁变形影响. 基于正态分布叠加原理的高速磁浮线路不平顺拟合方法,获得高速磁浮轨道不平顺的数值.
假定沿线路纵向布置的各长定子端部、功能件端部、桥墩高度、桥梁挠度在多种因素(如制造误差、温度引起非均匀变形,动载荷引起局部变形等)共同作用下的几何偏差为随机分布,且偏差值符合线路技术条件中的尺寸公差要求.
假设桥梁为受均匀分布载荷作用的欧拉-伯努利梁,在已知跨中挠度条件下,桥梁形变函数如式(5)所示[20].
{\textit{z}}(x) = \frac{{5{L^4}}}{{384{{\textit{z}}_{\text{m}}}}}\left(\frac{{{x^4}}}{{24}} - \frac{{L{x^3}}}{{12}} + \frac{{{L^3}x}}{{24}}\right), (5) 式中:x为桥梁长度方向的位置;zm为桥梁形变量;L为桥梁跨长.
以技术条件中不同部件端部公差值为
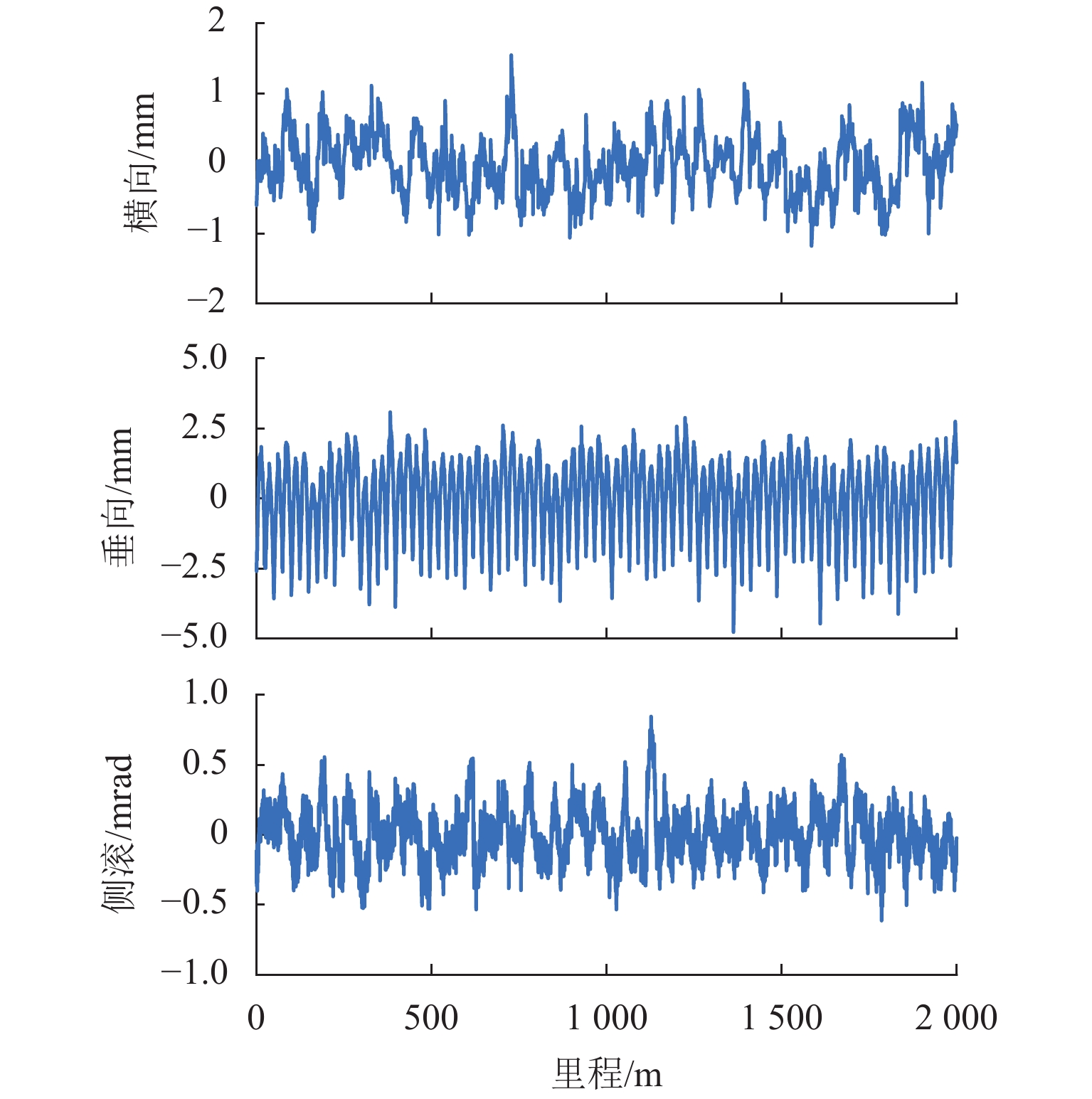
3\sigma 限值,以名义值作为均值,生成符合正态分布的一系列离散值,对离散值进行线性插值,再叠加式(6)中的桥梁形变函数,即可生成符合线路技术条件的轨道不平顺数据,拟合的横向、垂向和侧滚轨道不平顺见图8.3. 分析对比
根据磁浮车辆结构前后对称性以及曲线通过端部导向电磁铁受力最大,故选取原理样车二位端第一个控制器单元对应的导向、悬浮电磁铁为研究对象,分析曲线通过时刚性和柔性悬浮架对电磁铁的电磁力、间隙的影响.
同时,还开展了电磁铁间隙试验与仿真结果对比,分析了考虑悬浮架柔性特征的电磁铁动态运动更符合现车实际运动状态. 另外,还对电磁铁振动特性进行了分析,讨论了振动耦合效应.
3.1 电磁力
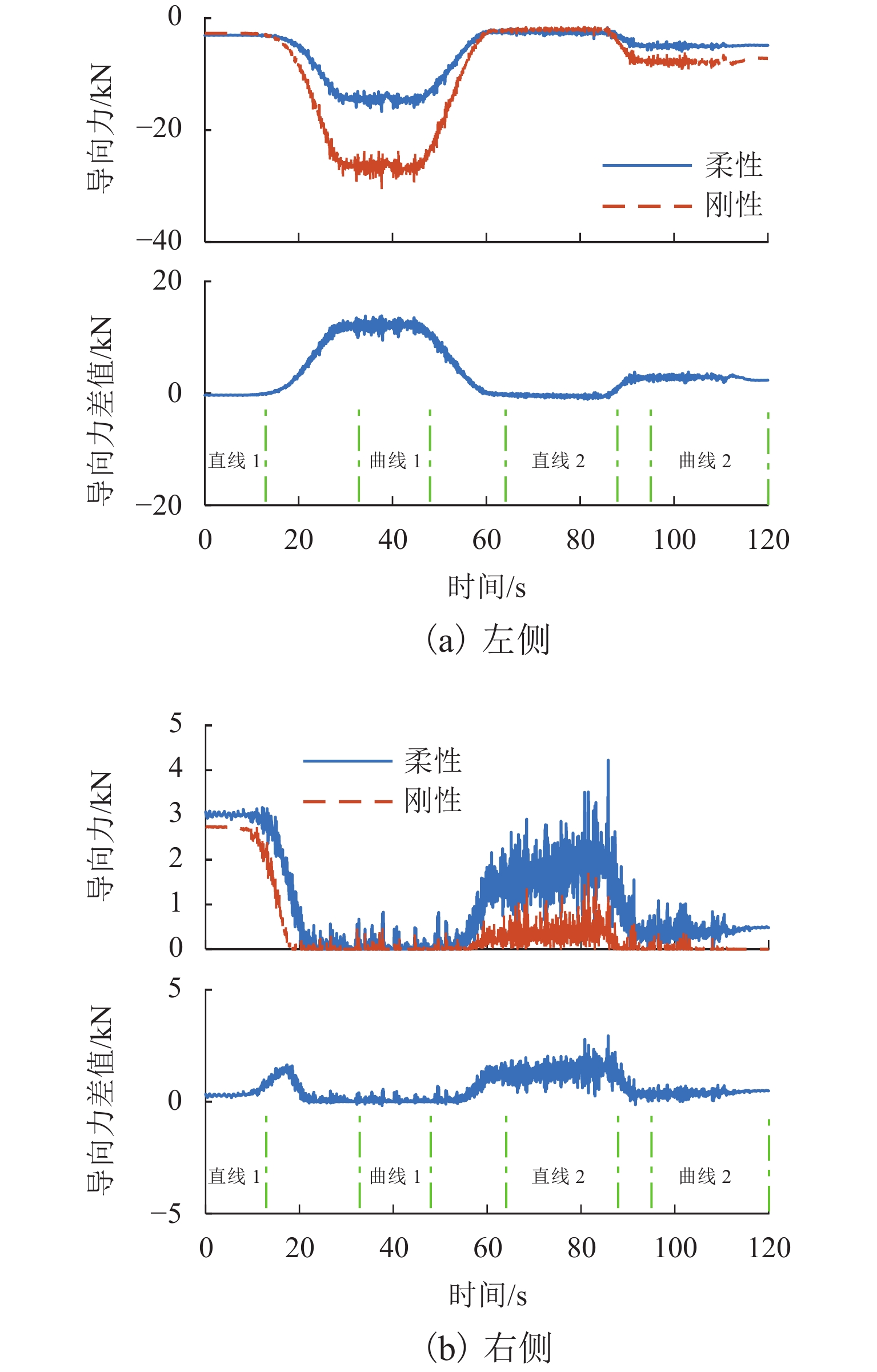
电磁铁名义工作点的电磁力大小及其动态变化范围决定着高速磁浮列车是否能够静态悬浮和沿轨道走行,也决定着电磁铁的振动状态. 高速磁浮车辆曲线通过时,其导向电磁铁的电磁力(简称导向力)起着重要作用,但每个电磁铁的前后半个电磁铁所需要的导向力也不尽相同,决定着导向电磁铁的磁极分布及配置.
图9给出了左右两侧导向力的时间历程,从图中可以看出:曲线通过时悬浮架柔性特征对左侧导向力的影响最为明显,尤其是通过小曲线时,两种悬浮架模型对应的导向力相差12.5 kN左右,刚性模型高估了曲线通过所需要的横向力.
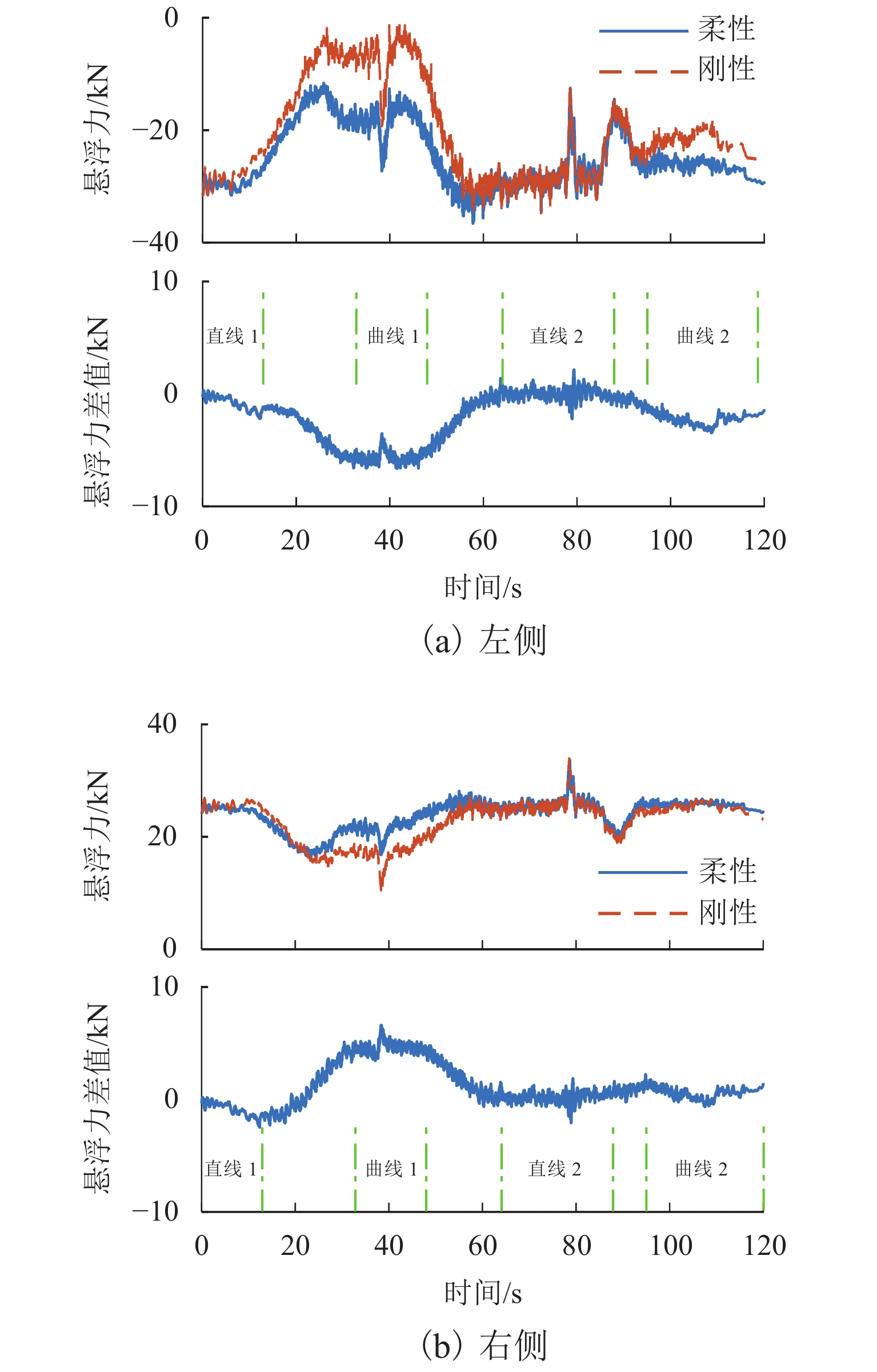
图10给出了左右两侧悬浮电磁铁电磁力(简称悬浮力)的时间历程,从图中可以看出:小曲线通过时悬浮架柔性特征对左右两侧悬浮力的影响最为明显,两种悬浮架模型对应的悬浮力相差6 kN左右,体现了小曲线通过时悬浮力一侧增载、另一侧减载的特征,且变化量接近.
3.2 间 隙
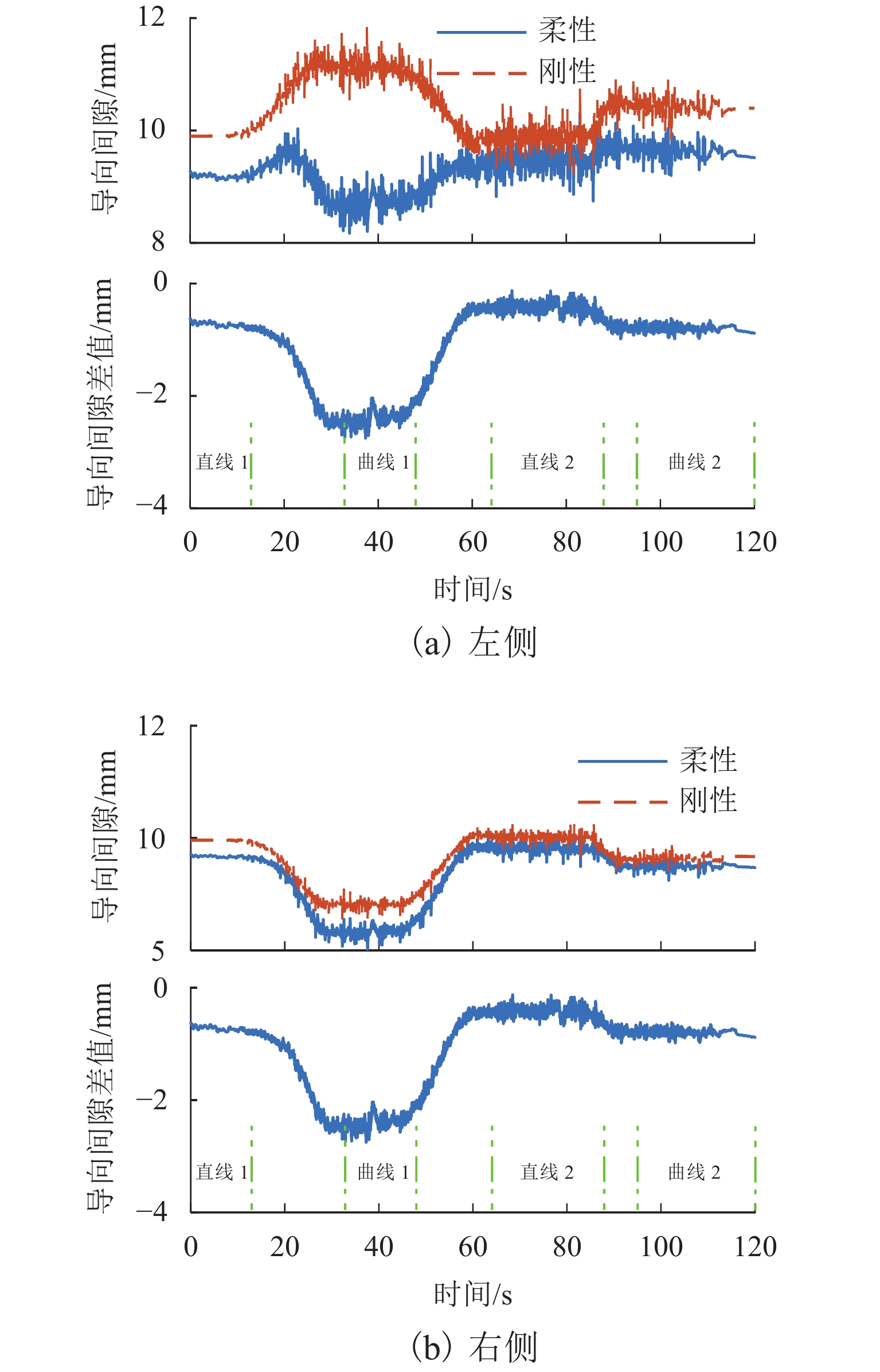
电磁铁间隙是常导磁浮车辆必须关注和考量的一个重要指标,反映了电磁铁跟随轨道运行状态的好坏,体现了电磁铁控制系统的鲁棒性和自适应性. 为了保护轨道上的长定子及其附属部件,车辆在运行过程中是不允许悬浮电磁铁触碰长定子的,而允许导向电磁铁可以碰撞导向板.
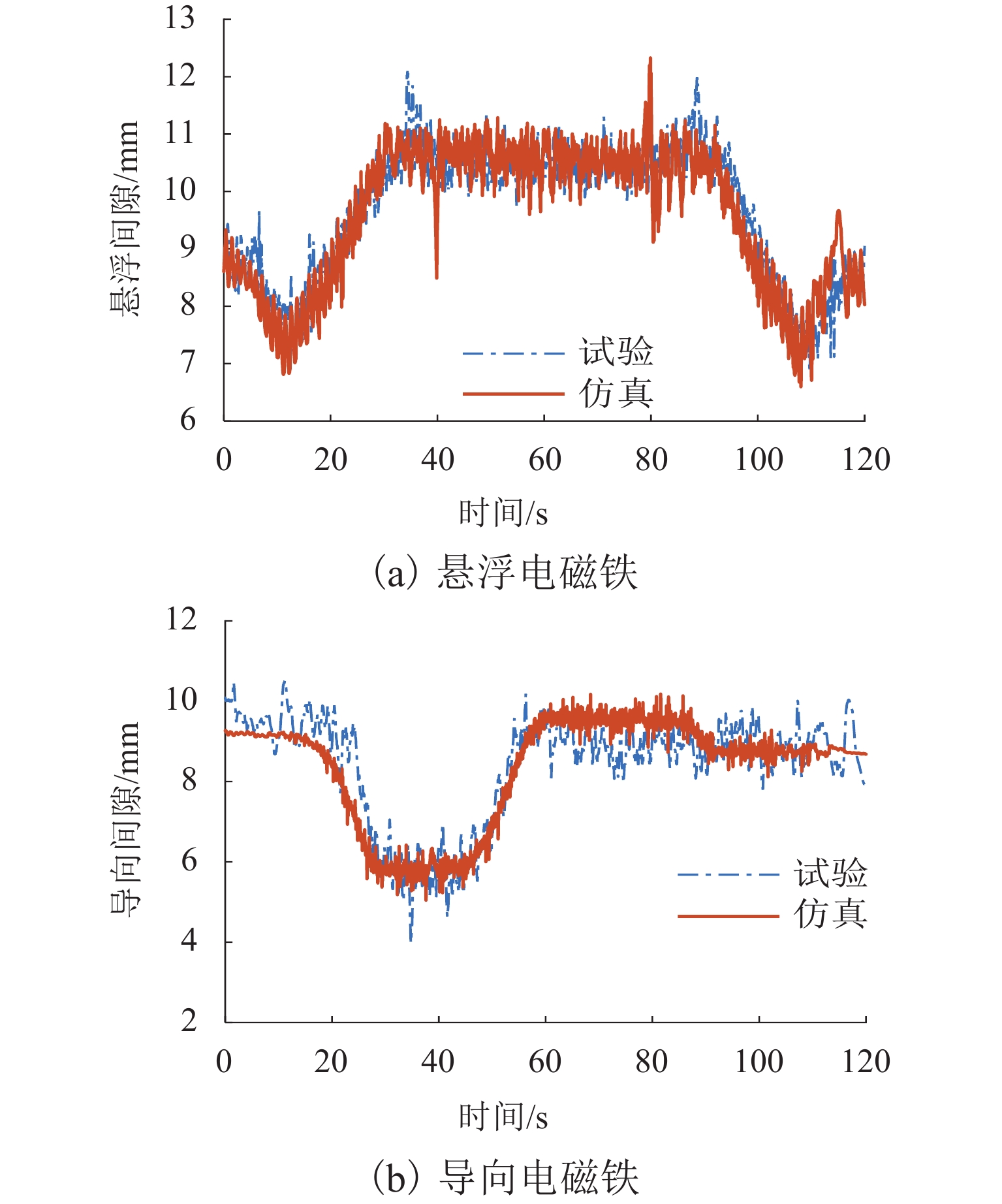
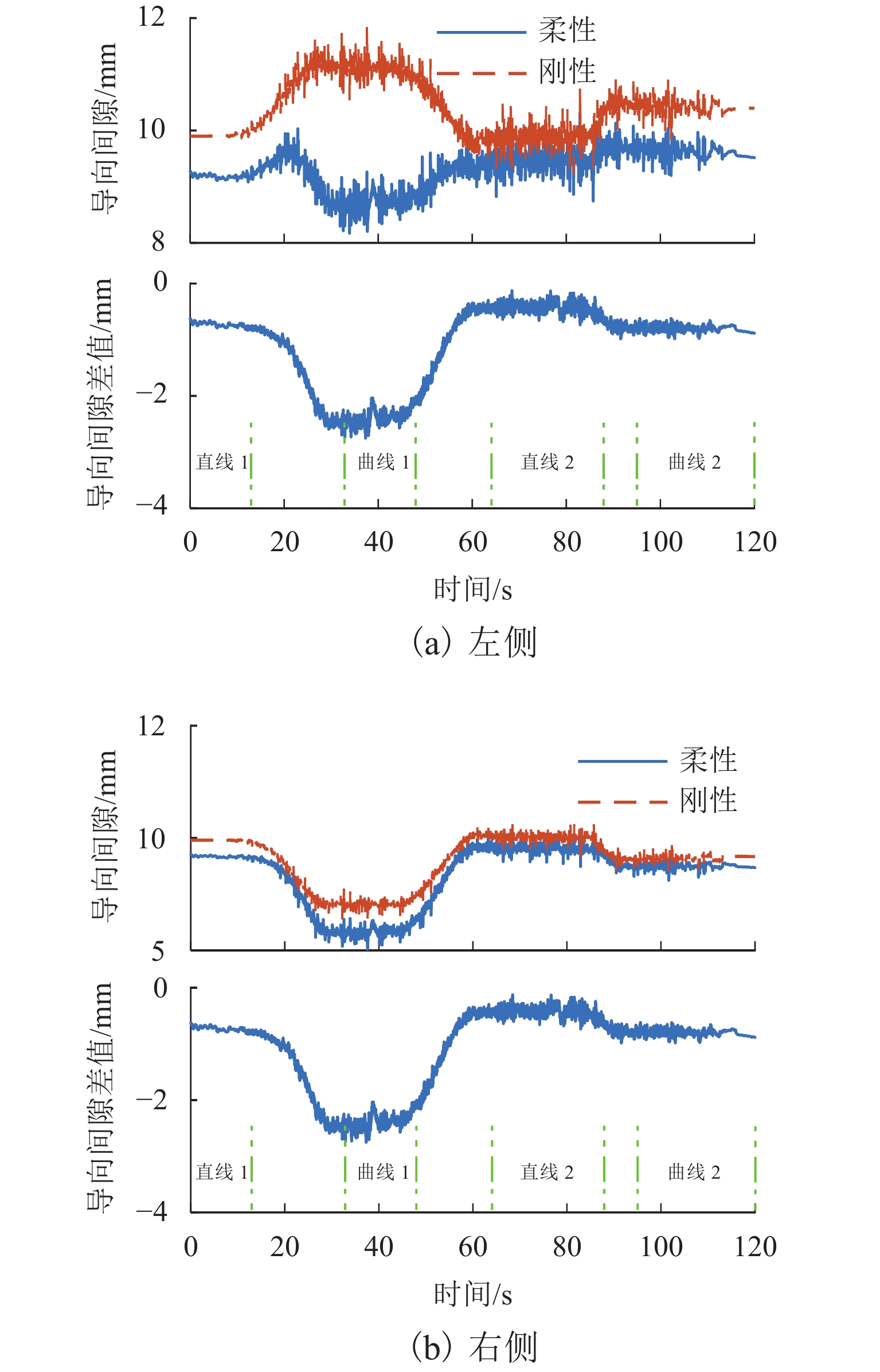
图11给出了左右两侧导向电磁铁间隙(简称导向间隙)的时间历程,从图中可以看出:若不考虑悬浮架的柔性振动,曲线通过时左右导向间隙符合刚体运动特征规律,即导向间隙一侧增大,另一侧则对应减小. 但是考虑悬浮架柔性振动时,悬浮架自身弹性变形使得上述特征规律变得并没有那么明显,其表现出的是两侧导向间隙均减小,靠近轨道中心线. 从图11中还可以看出:在车辆静止时,柔性模型产生了约0.60 mm的横向变形,使得左右导向间隙均小于刚性模型的,所以在控制系统中要考虑悬浮架结构变形产生的形变量. 在曲线1上,刚性和柔性悬浮架模型使右侧导向间隙差值达到1.60 mm.
图12给出了左右两侧悬浮电磁铁间隙(简称悬浮间隙)的时间历程,从图中可以看出:刚性和柔性悬浮架模型的悬浮间隙差值相近,并在0附近上下波动,最大相差0.75 mm (直线2),可能与轨道不平顺相关.
3.3 试验仿真对比
为了验证考虑悬浮架柔性特征的高速磁浮整车系统耦合动力学模型的准确性,将现场试验采集的电磁铁间隙信号与仿真模型同位置的计算结果进行对比,见图13,从图中可以看出:同一工况,仿真得到悬浮间隙和导向间隙分别与现车相同位置实测间隙数据相吻合. 说明目前所建立的高速磁浮车辆仿真模型具有较高的可信度,可以基于此模型开展高速运行时的动力学性能评估及其他相关研究工作.
3.4 振动特征
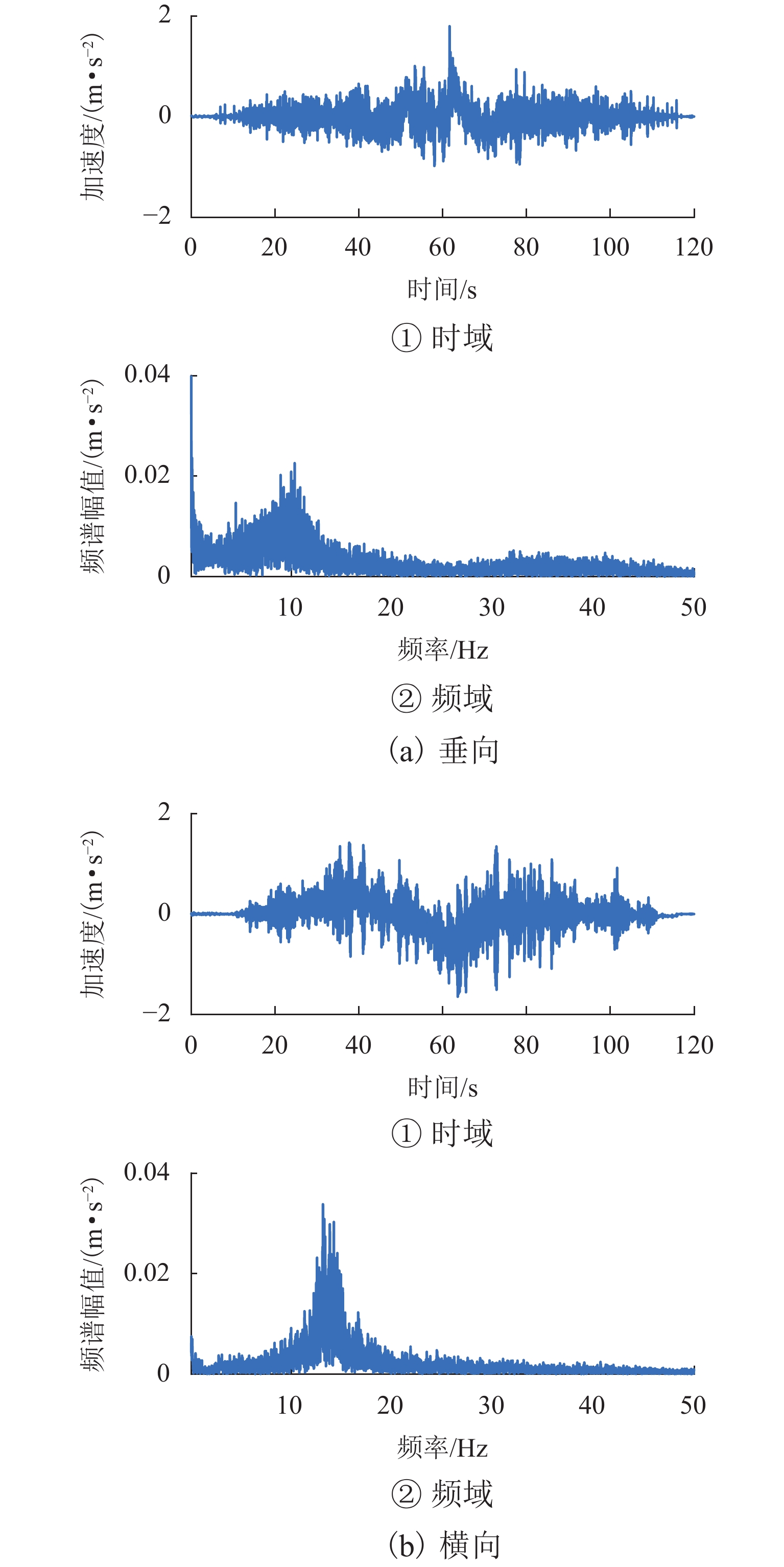
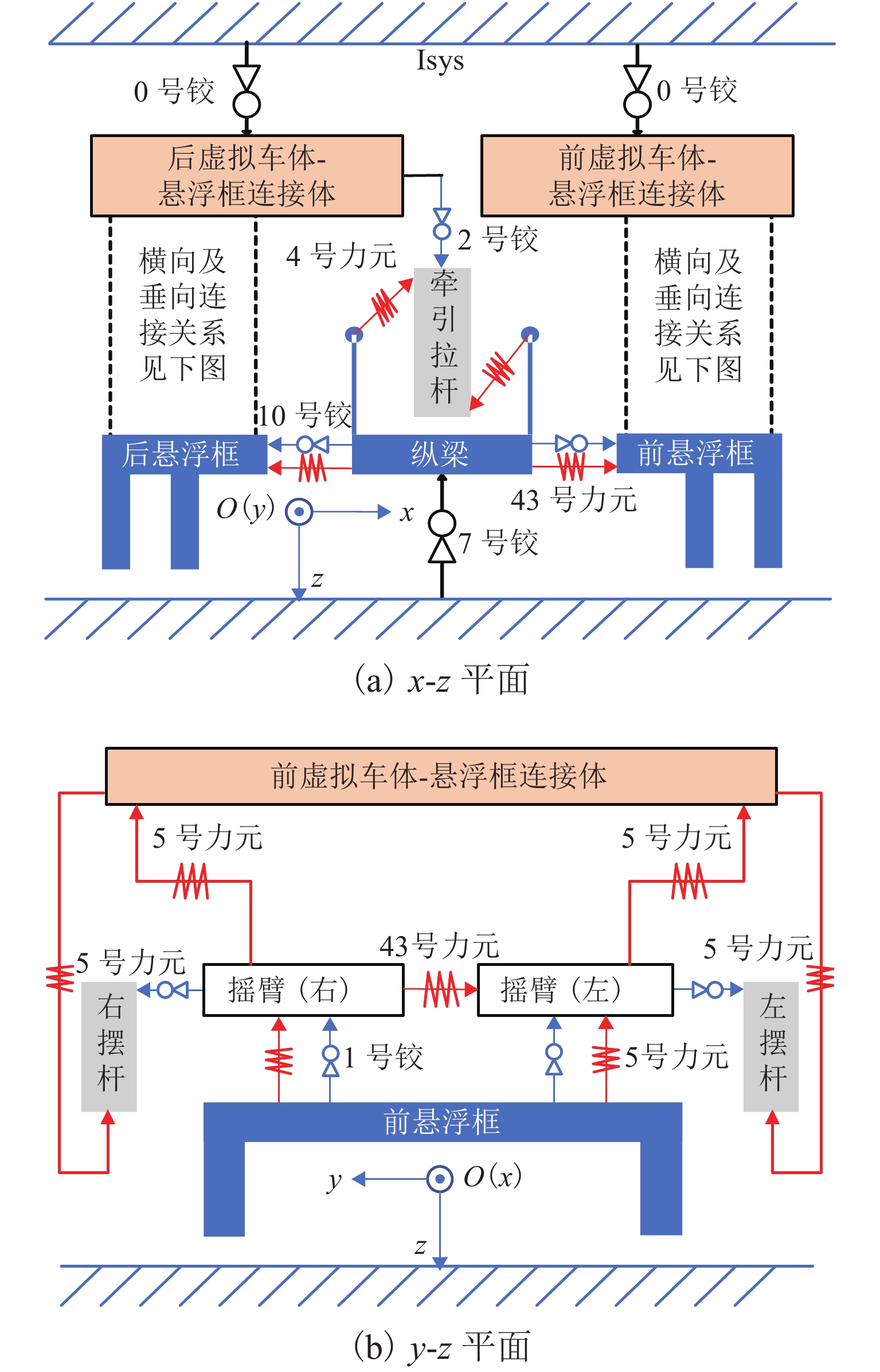
轨道不平顺激励从轨道传递给悬浮架后会激发起高速磁浮车辆系统的随机振动,为此,分别对悬浮架的横向和垂向加速度时域信号进行频谱分析,研究悬浮架运动过程中相关振动频率成分的来源. 从图14中可以看出:悬浮架垂向振动的主频为10.4 Hz,经对比,其与前后悬浮框相对点头的模态频率相近,可以认为此振动是由悬浮框相对点头振型引起的;而横向振动主频为13.2 Hz,与前后悬浮框反相摇头模态有关,其振动相关性较高.
4. 结 论
1) 高速磁浮车辆小曲线通过时,电磁铁动力学性能受悬浮架柔性振动的影响较大;通过试验仿真对比分析,考虑悬浮架柔性的计算结果更接近于实测结果.
2) 在研究控制参数优化、悬挂参数优化、运行稳定性、曲线通过能力等高速磁浮关键问题时应考虑悬浮架的弹性振动,不能忽视其影响.
3) 在磁浮车辆动力学模型验证、评估悬浮架结构强度和疲劳所需的悬浮架接口载荷计算时,可将悬浮架考虑成刚性模型.
-
表 1 高速磁浮整车动力学主要参数
Table 1. Main parameters in dynamics of high-speed maglev vehicles
部件 物理量 数值 悬浮电磁铁 质量/kg 600 侧滚转动惯量/(kg·m2) 1.5 点头转动惯量/(kg·m2) 479.8 摇头转动惯量/(kg·m2) 480.7 导向电磁铁 质量/kg 390 侧滚转动惯量/(kg·m2) 3.1 点头转动惯量/(kg·m2) 0.3 × 103 摇头转动惯量/(kg·m2) 0.3 × 103 悬浮架 质量/kg 1.7 × 103 侧滚转动惯量/(kg·m2) 3.5 × 103 点头转动惯量/(kg·m2) 4.4 × 103 摇头转动惯量/(kg·m2) 7.4 × 103 车体 质量/kg 3.0 × 104 侧滚转动惯量/(kg·m2) 5.7 × 104 点头转动惯量/(kg·m2) 1.6 × 106 摇头转动惯量/(kg·m2) 1.6 × 106 悬浮电磁铁安装刚度 纵向/(N·m−1) 1.0 × 107 横向/(N·m−1) 4.8 × 105 垂向/(N·m−1) 1.2 × 107 侧滚刚度/(N·m·rad−1) 1.0 × 107 点头刚度/(N·m·rad−1) 1.0 × 107 摇头刚度/(N·m·rad−1) 1.6 × 103 导向电磁铁安装刚度 纵向/(N·m−1) 4.3 × 107 横向/(N·m−1) 2.5 × 105 垂向/(N·m−1) 4.4 × 107 侧滚刚度/(N·m·rad−1) 1.0 × 106 点头刚度/(N·m·rad−1) 1.0 × 106 摇头刚度/(N·m·rad−1) 1.0 × 106 空气弹簧 垂向刚度/(N·m−1) 2.0 × 105 横向刚度/(N·m−1) 1.1 × 105 辅助弹簧 横向刚度/(N·m−1) 3.0 × 105 限位弹簧 横向刚度/(N·m−1) 5.0 × 105 摆杆 垂向刚度/(N·m−1) 2.0 × 105 侧滚刚度/(N·m·rad−1) 1.0 × 104 表 2 同济大学高速磁浮试验线主要参数表
Table 2. Main parameters about Tongji University’s maglev test line
区间 长度/m 横坡角/(°) 曲线半径/m 坡度/‰ 直线 1 234 0 ∞ 0 曲线 1 443 3 400 0 曲线 2 340 2 1300 0 直线 2 550 0 ∞ 11.2 -
[1] CUI Y X, SHEN G, WANG H. Maglev vehicle-guideway coupling vibration test rig based on the similarity theory[J]. Journal of Vibration and Control, 2016, 22(1): 286-295. doi: 10.1177/1077546314521446 [2] 武建军,沈飞,史筱红. 磁悬浮控制系统的稳定性及Hopf分岔的研究[J]. 振动与冲击,2010,29(3): 193-196, 214. doi: 10.3969/j.issn.1000-3835.2010.03.047WU Jianjun, SHEN Fei, SHI Xiaohong. Stability and Hopf bifurcation of the maglev system[J]. Journal of Vibration and Shock, 2010, 29(3): 193-196, 214. doi: 10.3969/j.issn.1000-3835.2010.03.047 [3] 梁鑫. 磁浮列车车轨耦合振动分析及试验研究[D]. 成都: 西南交通大学, 2015. [4] WANG K R, LUO S H, MA W H, et al. Dynamic characteristics analysis for a new-type maglev vehicle[J]. Advances in Mechanical Engineering, 2017, 9(12): 168781401774541.1-168781401774541.10. [5] 汪科任,罗世辉,张继业. 磁悬浮控制器设计及静悬浮稳定性分析[J]. 西南交通大学学报,2017,52(1): 118-126. doi: 10.3969/j.issn.0258-2724.2017.01.017WANG Keren, LUO Shihui, ZHANG Jiye. Design of magnetic levitation controller and static stability analysis[J]. Journal of Southwest Jiaotong University, 2017, 52(1): 118-126. doi: 10.3969/j.issn.0258-2724.2017.01.017 [6] 汪科任,罗世辉,宗凌潇,等. 新型磁浮车动力学仿真分析[J]. 振动与冲击,2017,36(20): 23-29.WANG Keren, LUO Shihui, ZONG Lingxiao, et al. A dynamic simulation analysis of new maglev trains[J]. Journal of Vibration and Shock, 2017, 36(20): 23-29. [7] 宗凌潇. 时速140km新型中低速磁浮列车走行机构研究分析[D]. 成都: 西南交通大学, 2016 . [8] 赵春霞. EMS型高速磁浮列车导向动力学研究[D]. 长沙: 国防科学技术大学, 2014. [9] 贺光. EMS型中速磁浮列车动力学建模与导向能力研究[D]. 长沙: 国防科学技术大学, 2016. [10] 谢钦. 新型中低速磁浮车辆空气弹簧应用研究[D]. 成都: 西南交通大学, 2017. [11] ZHANG M, LUO S H, GAO C, et al. Research on the mechanism of a newly developed levitation frame with mid-set air spring[J]. Vehicle System Dynamics, 2018, 56(12): 1797-1816. doi: 10.1080/00423114.2018.1435892 [12] ZHANG M, GAO C, MA W H. Effect of different connection modes of electromagnets on the performance of levitation control[J]. Proceedings of the Institution of Mechanical Engineers, Part F: Journal of Rail and Rapid Transit, 2018, 232(8): 2111-2125. doi: 10.1177/0954409718762171 [13] 闫一凡,齐洪峰,罗林涛,等. 基于UM的高速磁浮车辆刚柔耦合建模及振动传递规律研究[J]. 铁道机车车辆,2019,39(5): 59-64, 126.YAN Yifan, QI Hongfeng, LUO Lintao, et al. Rigid-flexible coupled modeling of high-speed maglev vehicle and vibration transmitting research base on UM[J]. Railway Locomotive & Car, 2019, 39(5): 59-64, 126. [14] 赵春发,翟婉明,叶学艳. 高速磁浮车辆弹性悬浮架动力学建模与仿真[J]. 系统仿真学报,2008,20(20): 5718-5721.ZHAO Chunfa, ZHAI Wanming, YE Xueyan. Dynamic modeling and simulation of high-speed maglev vehicle and its elastic levitation chassis[J]. Journal of System Simulation, 2008, 20(20): 5718-5721. [15] 叶学艳. 磁浮车辆系统动力学建模与仿真分析[D]. 成都: 西南交通大学, 2007. [16] DELLNITZ M, DIGNATH F, FLAßKAMP K, et al. Modelling and analysis of the nonlinear dynamics of the transrapid and its guideway[C]//Progress in Industrial Mathematics at ECMI 2010. Berlin: Springer, 2012: 113-123. [17] DING S S, SUN J J, HAN W T, et al. Modeling and analysis of a novel guidance magnet for high-speed maglev train[J]. IEEE Access, 2019, 7: 133324-133334. doi: 10.1109/ACCESS.2019.2940728 [18] 陈志贤. 高速常导电磁悬浮车辆系统动力学优化研究[D]. 成都: 西南交通大学, 2020. [19] 王军, 马云双. 中国高速动车组发展模式探索与实践[M]. 北京: 中国铁道出版社有限公司, 2020: 314-315. [20] 梁鑫,丁叁叁,黄超,等. 基于正态分布叠加原理的高速磁浮线路不平顺预测方法[J]. 机车电传动,2020(6): 20-24.LIANG Xin, DING Sansan, HUANG Chao, et al. Prediction method of irregularity of high-speed maglev track based on normal distribution superposition principle[J]. Electric Drive for Locomotives, 2020(6): 20-24. 期刊类型引用(3)
1. 卜秀孟,王力东,黎清蓉,胡朋,韩艳. 高速磁浮车-桥耦合振动控制参数影响分析. 西南交通大学学报. 2024(04): 848-857+866 .  本站查看
本站查看2. 付善强,梁鑫,丁叁叁. 高速磁浮车-轨耦合系统动态机理与振动响应. 同济大学学报(自然科学版). 2023(03): 314-320+384 .  百度学术
百度学术3. 付善强,吴冬华,韩伟涛,周颖. 基于非线性材料的高速磁浮电磁铁建模与分析. 西南交通大学学报. 2023(04): 879-885 .  本站查看
本站查看其他类型引用(2)
-






 下载:
下载:




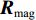
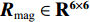

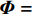


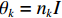
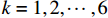
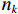

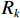



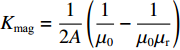
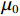
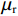
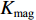
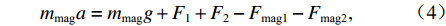
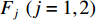
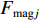
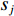



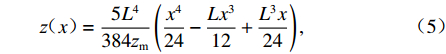
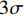







 下载:
下载:








 百度学术
百度学术