检修车轨道位置与导流板对扁平箱梁涡振的影响

黄林; 董佳慧; 王骑; 廖海黎

doi:10.3969/j.issn.0258-2724.20210474

检修车轨道位置与导流板对扁平箱梁涡振的影响

doi: 10.3969/j.issn.0258-2724.20210474

黄林^{1, 2,},
董佳慧^{1, 2},
王骑^{1, 2, ,},
廖海黎^{1, 2}

1.
西南交通大学风工程试验研究中心，四川成都 610031
2.
西南交通大学土木工程学院，四川成都 610031

基金项目: 国家自然科学基金（51778547, 51678508）

详细信息

作者简介:
黄林（1994—），男，博士研究生，研究方向为桥梁与隧道工程，E-mail：huanglin@my.swjtu.edu.cn

通讯作者:
王骑（1980—），男，副教授，研究方向为大跨度桥梁抗风，E-mail：wangchee_wind@swjtu.edu.cn

中图分类号: U441.3
计量
- 文章访问数: 317
- HTML全文浏览量: 84
- PDF下载量: 25
- 被引次数: 0
出版历程
- 收稿日期: 2021-06-17
- 修回日期: 2021-09-23
- 网络出版日期: 2023-01-03
- 刊出日期: 2021-09-29

Influence of Maintenance Rail Position and Guide Vanes on Vortex-Induced Vibration Performance of Flat Box Girders

HUANG Lin^{1, 2
,},
DONG Jiahui^{1, 2},
WANG Qi^{1, 2
, ,},
LIAO Haili^{1, 2}

1.
Research Center for Wind Engineering, Southwest Jiaotong University, Chengdu 610031, China
2.
School of Civil Engineering, Southwest Jiaotong University, Chengdu 610031, China

摘要

摘要:
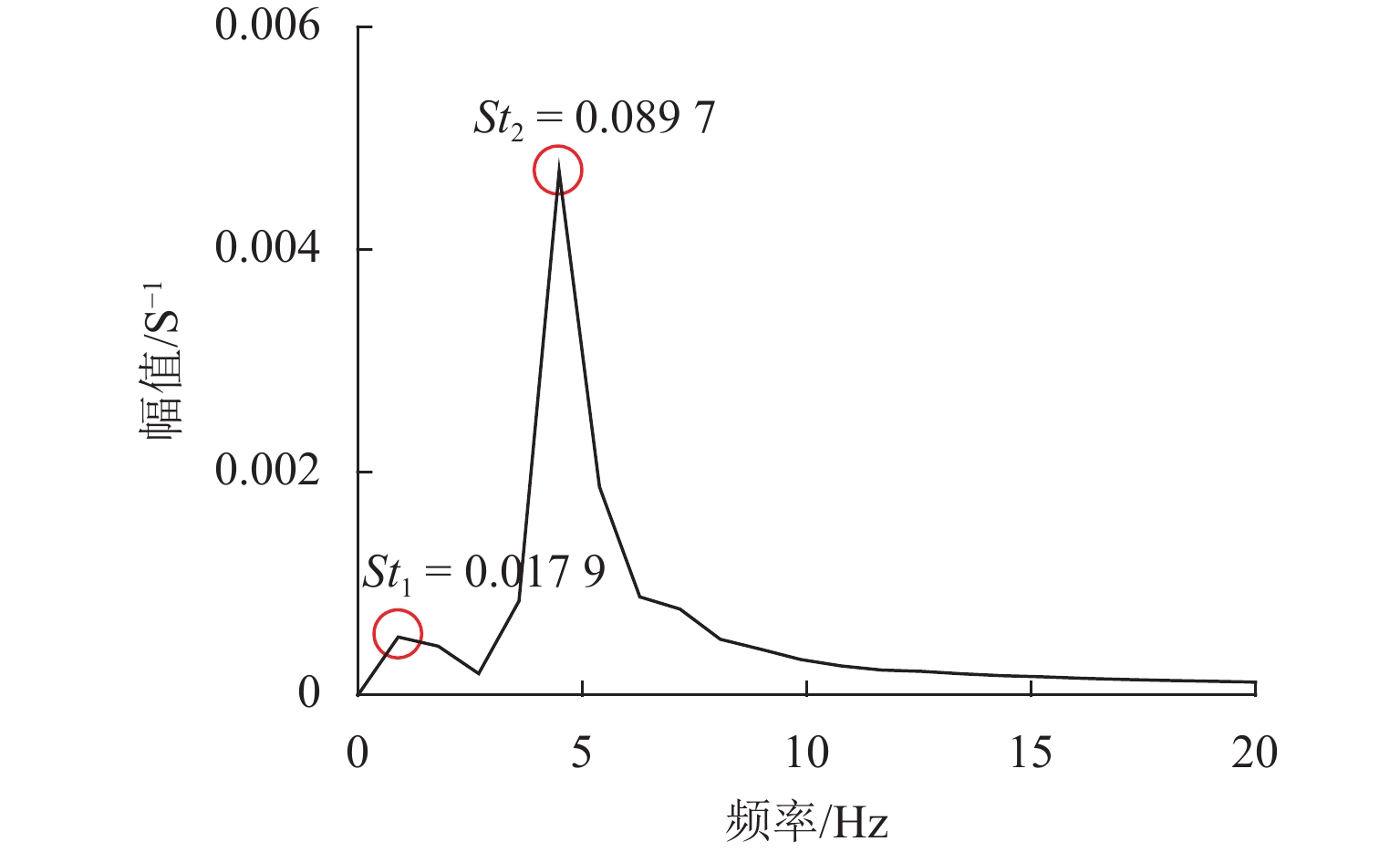
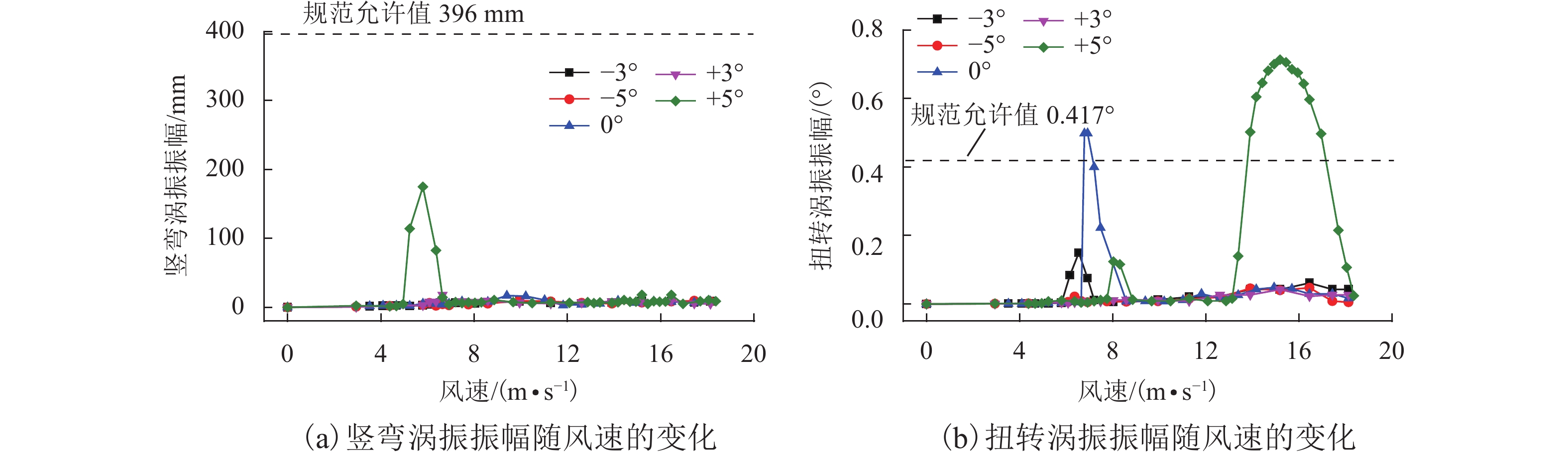
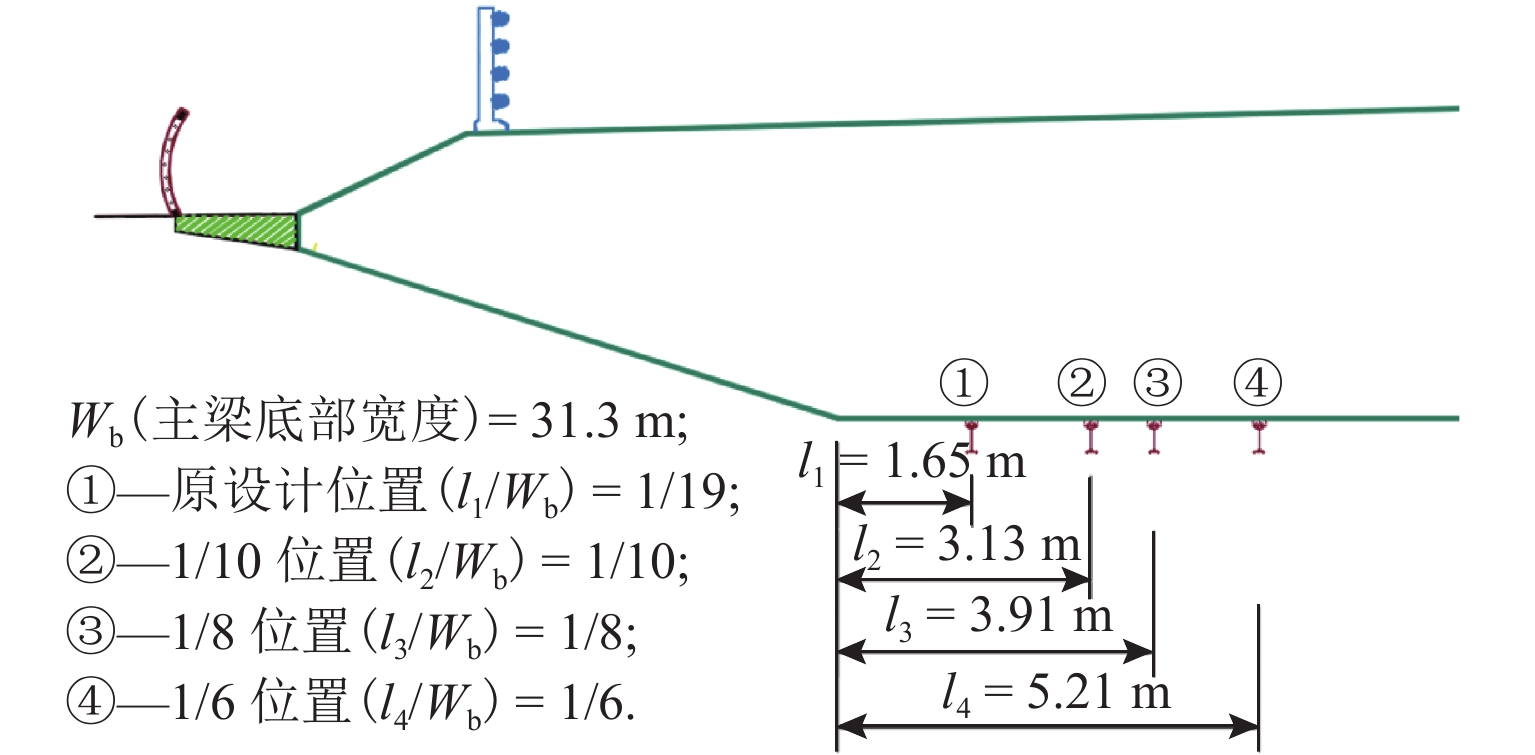
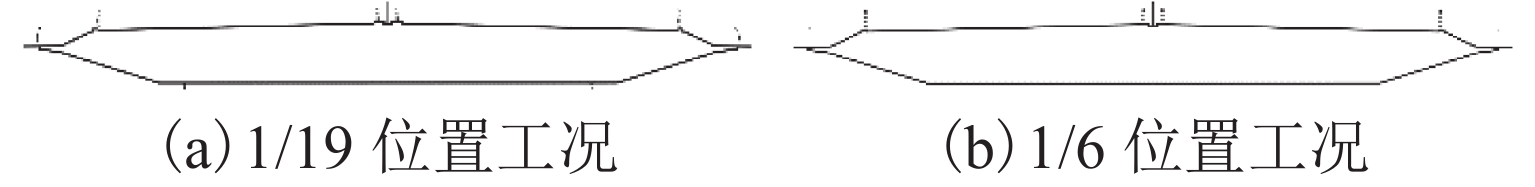
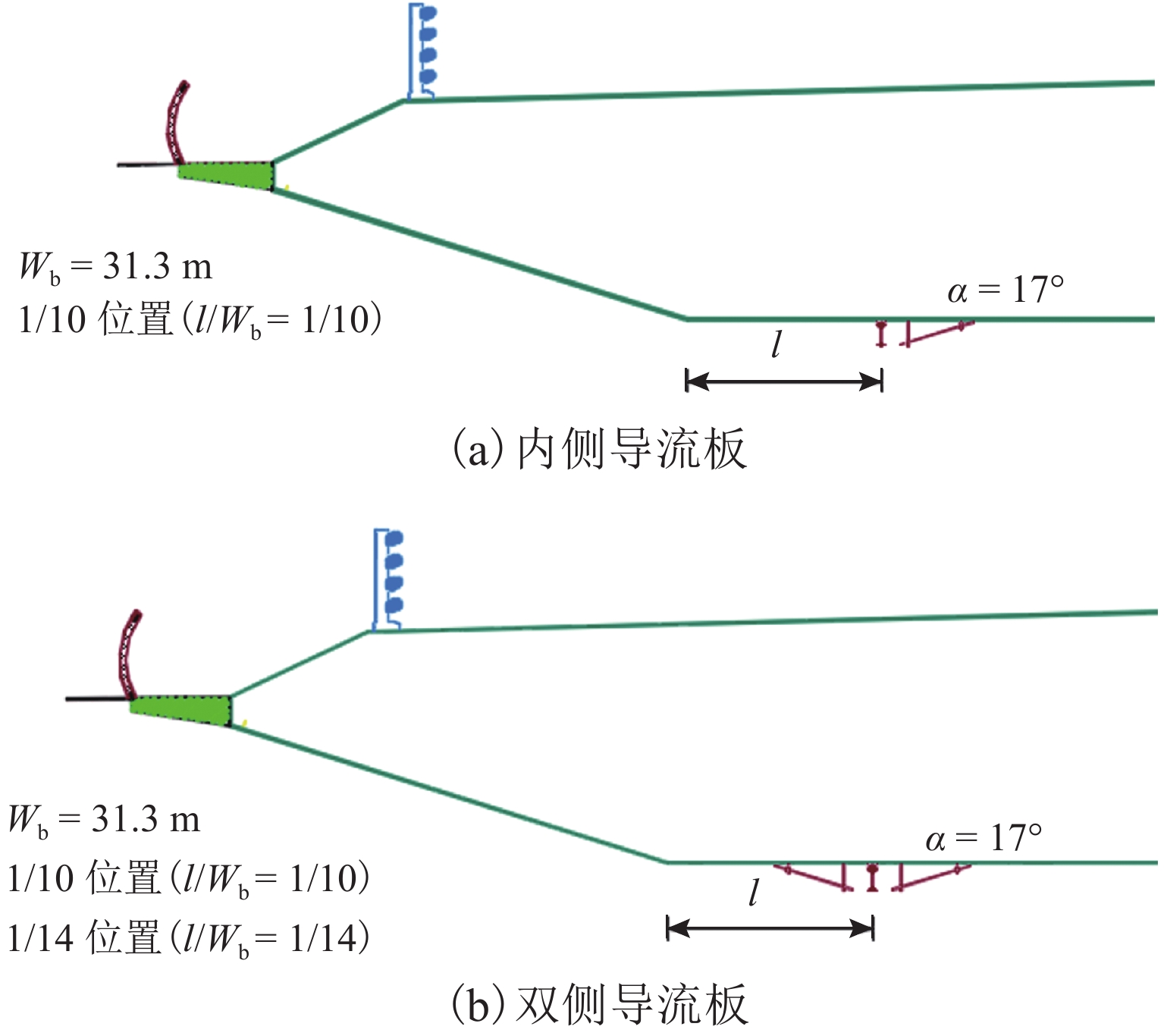
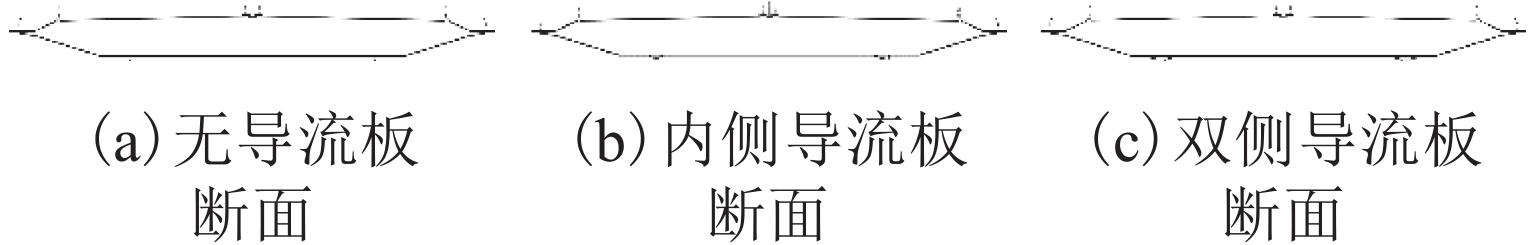
为研究检修车轨道位置与导流板对宽体扁平箱梁断面涡振性能的影响，以深中通道伶仃洋大桥（大跨度宽体扁平钢箱梁悬索桥）为背景，通过1∶25节段模型风洞试验测试了主梁的涡振响应，并采用计算流体动力学方法（CFD）对断面的二维流场进行了模拟. 结果表明：增大检修车轨道与主梁底板边缘之间距离l能够显著提高宽体扁平钢箱梁的涡振性能，当$l\geqslant {W}_{{\rm{b}}}/6$（${W}_{{\rm{b}}} $为主梁底部宽度）时，可完全消除宽体扁平箱梁在各风攻角下的涡激振动；在检修车轨道处设置17° 倾角的内侧或双侧导流板均能够显著抑制梁体的涡激振动，且抑制效果相同，当$l \geqslant {W}_{{\rm{b}}}/10$时，布置导流板可完全消除梁体的涡激振动；增大检修车轨道与主梁底板边缘之间距离以及设置导流板均是通过消除断面下游斜腹板处的尾流漩涡，从而降低梁体受到的周期性涡激力，达到抑制主梁涡振的效果.
- 宽体扁平钢箱梁 /
- 涡激振动 /
- 气动措施 /
- 检修车轨道 /
- 计算流体动力学（CFD） /
- 大比例尺节段模型
Abstract:
To study the influence of the maintenance rail position and the guide vanes on the vortex-induced vibration (VIV) performance of the wide flat box girder section, the Lingdingyang Bridge (a long-span suspension bridge with wide flat steel box girder) is taken as an example. The VIV of the main girder is studied using a 1∶25 scale section model wind tunnel test, and the two-dimensional flow field of the cross section is simulated using computational fluid dynamics. The test results show that increasing l (the distance between the maintenance rail and the bottom edge of the main girder) can significantly improve the VIV performance of a wide flat box girder. When $l\geqslant {W}_{{\rm{b}}}/6$ ($ {W}_{{\rm{b}}} $ is the bottom width of the main girder), the VIV of the girder can be completely eliminated under different wind attack angles. Installing a guide vane with a 17° angle inside or on both sides of the maintenance rail can significantly suppress the VIV of the girder, and the suppression effect is the same. When $l\geqslant {W}_{{\rm{b}}}/10$, the installation of the guide vane can completely eliminate the VIV of the girder. The numerical simulation results show that increasing the distance between the maintenance rail and the bottom edge of the girder and setting a guide vane can both significantly reduce the periodic vortex-induced force of the main girder by eliminating the wake vortex at the inclined web downstream of the section, thereby suppressing the VIV of the girder.
- wide flat steel box girder /
- vortex-induced vibration /
- aerodynamic countermeasures /
- maintenance rail /
- computational fluid dynamics (CFD) /
- large scale section model

HTML全文

图 1 伶仃洋大桥桥型布置（单位：m）

Figure 1. Layout of Lingdingyang bridge （unit：m）

下载: 全尺寸图片幻灯片

图 2 伶仃洋大桥主梁（单位：m）

Figure 2. Lingdingyang bridge girder （unit：m）

下载: 全尺寸图片幻灯片

图 3 节段模型

Figure 3. Section model in wind tunnel

下载: 全尺寸图片幻灯片

图 4 原设计断面主梁涡振响应

Figure 4. Vortex-induced vibration displacement of the original section

下载: 全尺寸图片幻灯片

图 5 检修车轨道位置示意

Figure 5. Diagram of maintenance rail position

下载: 全尺寸图片幻灯片

图 6 计算断面

Figure 6. Calculated cross-section

下载: 全尺寸图片幻灯片

图 7 CFD计算域

Figure 7. CFD computational domain

下载: 全尺寸图片幻灯片

图 8 1/19位置工况断面${C}_{{\rm{L}}}\left(t\right)$频谱

Figure 8. ${C}_{{\rm{L}}}\left(t\right)$ spectrum of section with maintenance rail on the 1/19 position

下载: 全尺寸图片幻灯片

图 9 检修车轨道各位置工况断面涡量与迹线图

Figure 9. Vorticity magnitude and trace diagram around the section with different maintenance rail positions

下载: 全尺寸图片幻灯片

图 10 数值模拟静力三分力时程图

Figure 10. Time-history diagram of the static coefficients in numerical simulation

下载: 全尺寸图片幻灯片

图 11 检修车轨道导流板示意

Figure 11. Diagram of the maintenance rail guide vane

下载: 全尺寸图片幻灯片

图 12 计算断面

Figure 12. Calculated cross-section

下载: 全尺寸图片幻灯片

图 13 各导流板工况断面涡量与迹线图

Figure 13. Vorticity magnitude and trace diagram around the section with different guide vanes

下载: 全尺寸图片幻灯片

图 14 全桥气弹模型

Figure 14. Full bridge aeroelastic model

下载: 全尺寸图片幻灯片

表 1 节段模型试验参数取值

Table 1. Section model test parameters

参数名称	实桥值	相似比	模型值
等效质量/（kg•m⁻¹）	42156	1/25²	67.45
等效质量惯性矩/ （kg•m²•m⁻¹）	10007344	1/25⁴	25.62
竖弯频率/Hz	0.101	13.960	1.410
扭转频率/Hz	0.220	10.500	2.310
竖弯阻尼比/%	0.30	—	0.24
扭转阻尼比/%	0.30	—	0.10

下载: 导出CSV

表 2 检修车轨道各位置工况涡振幅值

Table 2. VIV displacement of each working condition with different maintenance rail positions

风攻角/（°）	竖弯涡振振幅/mm				扭转涡振振幅/（°）
风攻角/（°）	1/6 位置	1/8 位置	1/10 位置	1/19 位置	1/6 位置	1/8 位置	1/10 位置	1/19 位置
0	6.4	7.0	5.3	17.1	0.01	0.07	0.55	0.50
+3	6.9	7.9	6.4	18.1	0.02	0.01	0.02	0.04
+5	7.9	7.6	6.8	174.9	0.02	0.02	0.45	0.71
−3	6.5	7.7	7.5	8.5	0.01	0.21	0.02	0.15
−5	7.2	8.5	7.9	9.7	0.02	0.02	0.03	0.05

下载: 导出CSV

表 3 数值模拟参数设置

Table 3. Parameters of the numerical simulation

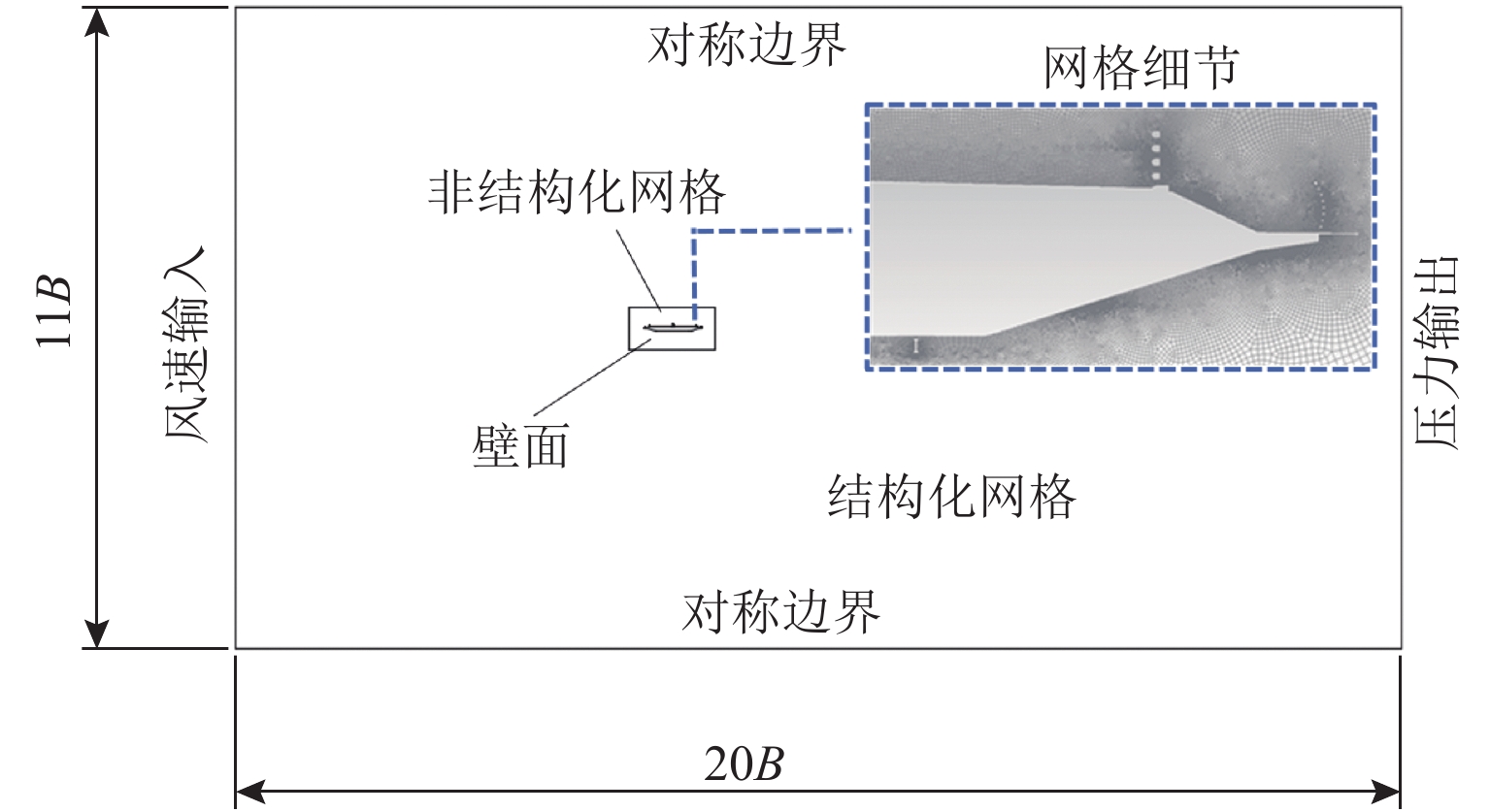
参数	湍流长度尺度/m	湍流强度/%	时间步长	算法
取值	0.08	0.5	0.00002	SIMPLE

下载: 导出CSV

表 4 各导流板工况涡振幅值

Table 4. VIV displacement of each working condition with different guide vanes

风攻角/（°）	竖弯涡振振幅/mm				扭转涡振振幅/（°）
风攻角/（°）	1/14 位置双侧导流板	1/10 位置双侧导流板	1/10 位置内侧导流板	1/10 位置无导流板	1/14 位置双侧导流板	1/10 位置双侧导流板	1/10 位置内侧导流板	1/10 位置无导流板
0	38.4	5.3	5.5	5.3	0.37	0.01	0.01	0.55
+3	6.9	6.4	6.3	6.4	0.02	0.01	0.02	0.02
+5	12.2	8.3	7.5	6.8	0.45	0.02	0.02	0.45
−3	5.9	8.4	8.6	7.5	0.02	0.01	0.01	0.02
−5	7.3	8.8	8.7	7.9	0.02	0.01	0.01	0.03

下载: 导出CSV

表 5 全桥气弹模型试验参数取值

Table 5. Full bridge aeroelastic model test parameters

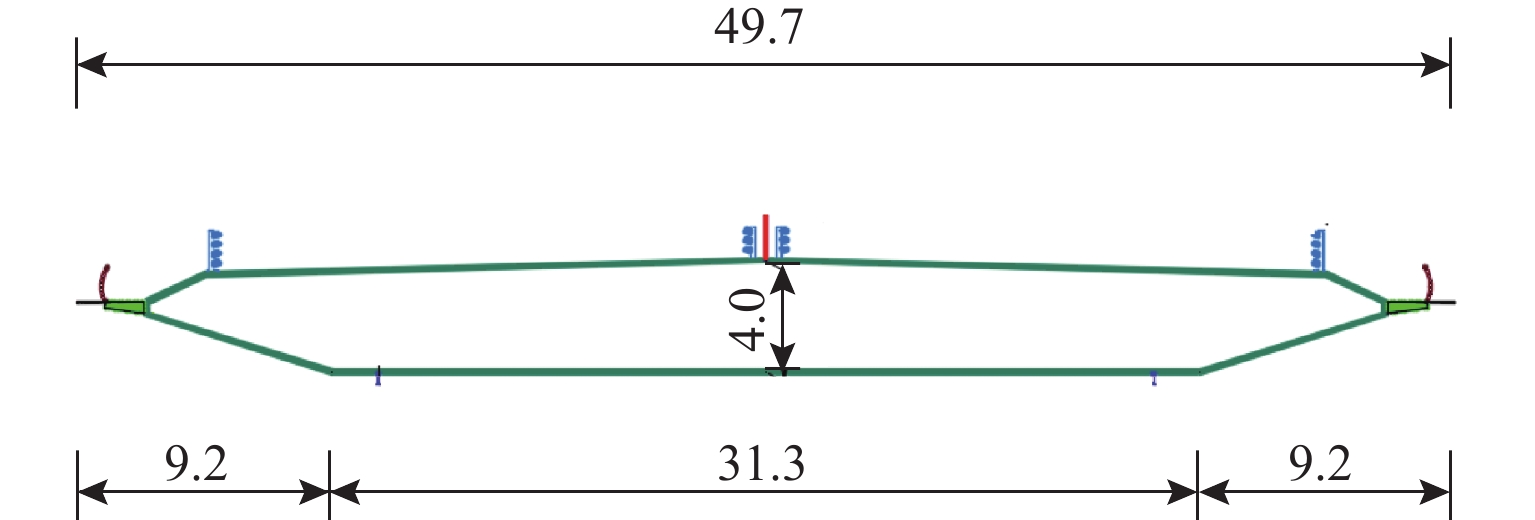
参数名称	实桥值	相似比	模型值
主梁长/m	1666	1/134	12.43
梁宽/m	49.7	1/134	0.371
梁高/m	4	1/134	0.030
单位长度质量/（kg•m⁻¹）	32496	1/134²	1.8098
单位长度质量惯性矩/ （kg•m²•m⁻¹）	5722181	1/134⁴	0.0177

下载: 导出CSV

表 6 全桥气弹模型模态参数

Table 6. Modal testing parameters of full bridge aeroelastic model

振型	实桥频率/Hz	模型频率			阻尼比/%
振型	实桥频率/Hz	要求值/Hz	实测值/Hz	误差/%	阻尼比/%
V-S-1	0.1010	1.169	1.092	5.70	0.34
V-A-1	0.0960	1.112	1.121	1.10	0.32
T-S-1	0.2196	2.542	2.569	2.30	0.34
T-A-1	0.2263	2.62	2.6321	1.80	0.40
L-S-1	0.0564	0.661	0.6601	1.50	0.23
L-A-1	0.1345	1.557	1.5622	1.50	0.28

下载: 导出CSV

参考文献(29)

[1]	政清. 桥梁风工程[M]. 北京: 人民交通出版社, 2005.
[2]	管青海,李加武,胡兆同,等. 栏杆对典型桥梁断面涡激振动的影响研究[J]. 振动与冲击,2014,33(3): 150-156. doi: 10.3969/j.issn.1000-3835.2014.03.029 GUAN Qinghai, LI Jiawu, HU Zhaotong, et al. Effects of railings on vortex-induced vibration of a bridge deck section[J]. Journal of Vibration and Shock, 2014, 33(3): 150-156. doi: 10.3969/j.issn.1000-3835.2014.03.029
[3]	李春光,陈政清,韩阳. 带悬挑人行道板流线型箱梁涡振性能研究[J]. 振动与冲击,2014,33(24): 19-25. doi: 10.13465/j.cnki.jvs.2014.24.004 LI Chunguang, CHEN Zhengqing, HAN Yang. Vortex induced vibration performance of a streamlined box girder with a cantilevered walking slab[J]. Journal of Vibration and Shock, 2014, 33(24): 19-25. doi: 10.13465/j.cnki.jvs.2014.24.004
[4]	杨阳,张亮亮,吴波,等. 宽体扁平钢箱梁气动力特性及涡振性能研究[J]. 桥梁建设,2016,46(1): 70-75. YANG Yang, ZHANG Liangliang, WU Bo, et al. Study of aerodynamic characteristics and vortex-induced vibration performance of wide flat steel box girder[J]. Bridge Construction, 2016, 46(1): 70-75.
[5]	LARSEN A, WALL A. Shaping of bridge box girders to avoid vortex shedding response[J]. Journal of Wind Engineering and Industrial Aerodynamics, 2012, 104/105/106: 159-165.
[6]	LARSEN A, ESDAHL S, ANDERSEN J E, et al. Storebælt suspension bridge-vortex shedding excitation and mitigation by guide vanes[J]. Journal of Wind Engineering and Industrial Aerodynamics, 2000, 88(2/3): 283-296.
[7]	NAGAO F, UTSUNOMIYA H, YOSHIOKA E, et al. Effects of handrails on separated shear flow and vortex-induced oscillation[J]. Journal of Wind Engineering and Industrial Aerodynamics, 1997, 69/70/71: 819-827.
[8]	CHEN Z S, LIU S M, YU X F, et al. Experimental investigations on VIV of bridge deck sections: a case study[J]. KSCE Journal of Civil Engineering, 2017, 21(7): 2821-2827. doi: 10.1007/s12205-017-0120-1
[9]	ZHAN J, XIN D B, OU J P, et al. Experimental study on suppressing vortex-induced vibration of a long-span bridge by installing the wavy railings[J]. Journal of Wind Engineering and Industrial Aerodynamics, 2020, 202(8): 104205.1-104205.13.
[10]	李浩弘,吴波,张亮亮,等. 基于风洞试验及大涡模拟的宽体扁平箱梁涡振特性研究与优化[J]. 建筑结构学报,2018,39(增1): 29-35. doi: 10.14006/j.jzjgxb.2018.S1.005 LI Haohong, WU Bo, ZHANG Liangliang, et al. Investigation and optimization on VIV performances of wide flat box girders based on wind tunnel tests and large eddy simulations[J]. Journal of Building Structures, 2018, 39(S1): 29-35. doi: 10.14006/j.jzjgxb.2018.S1.005
[11]	张建,郑史雄,唐煜,等. 基于节段模型试验的悬索桥涡振性能优化研究[J]. 实验流体力学,2015,29(2): 48-54. doi: 10.11729/syltlx20140037 ZHANG Jian, ZHENG Shixiong, TANG Yu, et al. Research on optimizing vortex-induced vibration performance for suspension bridge based on section model test[J]. Journal of Experiments in Fluid Mechanics, 2015, 29(2): 48-54. doi: 10.11729/syltlx20140037
[12]	刘君,廖海黎,万嘉伟,等. 检修车轨道导流板对流线型箱梁涡振的影响[J]. 西南交通大学学报,2015,50(5): 789-795. doi: 10.3969/j.issn.0258-2724.2015.05.004 LIU Jun, LIAO Haili, WAN Jiawei, et al. Effect of guide vane beside maintenance rail on vortex-induced vibration of streamlined box girder[J]. Journal of Southwest Jiaotong University, 2015, 50(5): 789-795. doi: 10.3969/j.issn.0258-2724.2015.05.004
[13]	李明,孙延国,李明水,等. 宽幅流线型箱梁涡振性能及制振措施研究[J]. 西南交通大学学报,2018,53(4): 712-719. doi: 10.3969/j.issn.0258-2724.2018.04.007 LI Ming, SUN Yanguo, LI Mingshui, et al. Vortex-induced vibration performance of wide streamlined box girder and aerodynamic countermeasure research[J]. Journal of Southwest Jiaotong University, 2018, 53(4): 712-719. doi: 10.3969/j.issn.0258-2724.2018.04.007
[14]	LI M, SUN Y G, JING H M, et al. Vortex-induced vibration optimization of a wide streamline box girder by wind tunnel test[J]. KSCE Journal of Civil Engineering, 2018, 22(12): 5143-5153. doi: 10.1007/s12205-018-0548-y
[15]	朱思宇,李永乐,申俊昕,等. 大攻角来流作用下扁平钢箱梁涡振性能风洞试验优化研究[J]. 土木工程学报,2015,48(2): 79-86. doi: 10.15951/j.tmgcxb.2015.02.012 ZHU Siyu, LI Yongle, SHEN Junxin, et al. Optimization of vortex-induced vibration of flat steel box girders at large attack angle by wind tunnel test[J]. China Civil Engineering Journal, 2015, 48(2): 79-86. doi: 10.15951/j.tmgcxb.2015.02.012
[16]	孙延国,廖海黎,李明水. 基于节段模型试验的悬索桥涡振抑振措施[J]. 西南交通大学学报,2012,47(2): 218-223,264. doi: 10.3969/j.issn.0258-2724.2012.02.008 SUN Yanguo, LIAO Haili, LI Mingshui. Mitigation measures of vortex-induced vibration of suspension bridge based on section model test[J]. Journal of Southwest Jiaotong University, 2012, 47(2): 218-223,264. doi: 10.3969/j.issn.0258-2724.2012.02.008
[17]	胡传新,赵林,周志勇,等. 流线型闭口箱梁抑流板抑制涡振机理研究[J]. 振动工程学报,2020,33(1): 1-11. doi: 10.16385/j.cnki.issn.1004-4523.2020.01.001 HU Chuanxin, ZHAO Lin, ZHOU Zhiyong, et al. Suppressing mechanism of spoilers on vortex-induced vibrations around a streamlined closed-box girder based on characteristics of aerodynamics forces and flow field[J]. Journal of Vibration Engineering, 2020, 33(1): 1-11. doi: 10.16385/j.cnki.issn.1004-4523.2020.01.001
[18]	HU C X, ZHAO L, GE Y J. Mechanism of suppression of vortex-induced vibrations of a streamlined closed-box girder using additional small-scale components[J]. Journal of Wind Engineering and Industrial Aerodynamics, 2019, 189: 314-331. doi: 10.1016/j.jweia.2019.04.015
[19]	SCHEWE G, LARSEN A. Reynolds number effects in the flow around a bluff bridge deck cross section[J]. Journal of Wind Engineering and Industrial Aerodynamics, 1998, 74/75/76: 829-838.
[20]	SCHEWE G. Reynolds-number effects in flow around more-or-less bluff bodies[J]. Journal of Wind Engineering and Industrial Aerodynamics, 2001, 89(14/15): 1267-1289.
[21]	胡传新,赵林,陈海兴,等. 流线闭口箱梁涡振气动力的雷诺数效应研究[J]. 振动与冲击,2019,38(12): 118-125. doi: 10.13465/j.cnki.jvs.2019.12.017 HU Chuanxin, ZHAO Lin, CHEN Haixing, et al. Reynolds number effects on aerodynamic forces of a streamlined closed-box girder during vortex-induced vibrations[J]. Journal of Vibration and Shock, 2019, 38(12): 118-125. doi: 10.13465/j.cnki.jvs.2019.12.017
[22]	SCANLAN R H. Observations on low-speed aeroelasticity[J]. Journal of Engineering Mechanics, 2002, 128(12): 1254-1258. doi: 10.1061/(ASCE)0733-9399(2002)128:12(1254)
[23]	李加武,张宏杰,韩万水. 斜拉桥风致响应的雷诺数效应[J]. 中国公路学报,2009,22(2): 42-47. doi: 10.3321/j.issn:1001-7372.2009.02.008 LI Jiawu, ZHANG Hongjie, HAN Wanshui. Wind-induced response of cable-stayed bridge with consideration of Reynolds number effect[J]. China Journal of Highway and Transport, 2009, 22(2): 42-47. doi: 10.3321/j.issn:1001-7372.2009.02.008
[24]	熊龙,孙延国,廖海黎. 钢箱梁在高低雷诺数下的涡振特性研究[J]. 桥梁建设,2016,46(5): 65-70. XIONG Long, SUN Yanguo, LIAO Haili. Study of vortex-induced vibration characteristics of steel box girder at high and low Reynolds numbers[J]. Bridge Construction, 2016, 46(5): 65-70.
[25]	董浩天,葛耀君,杨詠昕. 闭口钢箱梁悬索桥涡振多尺度模型风洞试验[J]. 结构工程师,2018,34(4): 94-100. doi: 10.3969/j.issn.1005-0159.2018.04.014 DONG Haotian, GE Yaojun, YANG Yongxin. Multi-scale model tests of vortex-induced vibration of a suspension bridge with closed steel box girders[J]. Structural Engineers, 2018, 34(4): 94-100. doi: 10.3969/j.issn.1005-0159.2018.04.014
[26]	任若松,梁新华,刘小兵,等. 准流线型桥梁断面气动力特性的雷诺数效应研究[J]. 工程力学,2020,37(增1): 139-144,167. REN Ruosong, LIANG Xinhua, LIU Xiaobing, et al. Study on Reynolds number effect of aerodynamic characteristics of quasi-streamlined bridge section[J]. Engineering Mechanics, 2020, 37(S1): 139-144,167.
[27]	交通运输部. 公路桥梁抗风设计规范: JTG/T 3360-01—2018[S]. 北京: 人民交通出版社, 2018.
[28]	MENTER F R. Two-equation eddy-viscosity turbulence models for engineering applications[J]. AIAA Journal, 1994, 32(8): 1598-1605. doi: 10.2514/3.12149
[29]	熊龙,廖海黎,李明水,等. 马普托桥颤振稳定性的风洞试验研究[J]. 防灾减灾工程学报,2017,37(2): 321-326. doi: 10.13409/j.cnki.jdpme.2017.02.021 XIONG Long, LIAO Haili, LI Mingshui, et al. Wind tunnel test study on flutter stability of Maputo bridge[J]. Journal of Disaster Prevention and Mitigation Engineering, 2017, 37(2): 321-326. doi: 10.13409/j.cnki.jdpme.2017.02.021