Optimal Design of Heavy-Haul Rail Grinding Profile Considering Grinding Amount
-
摘要:
为在重载钢轨打磨廓形优化设计中最小化钢轨打磨量,建立了打磨量的钢轨廓形对齐及计算方法,设计以轮轨磨耗指数、轮轨接触应力以及钢轨打磨量为优化子目标的综合优化评价模型,并对不同优化策略的优化结果进行了分析. 首先,通过矩阵旋转变换、曲线拟合及样条插值等理论建立钢轨廓形自动对齐算法,并计算目标廓形打磨量;其次,考虑轮轨磨耗指数、接触应力以及钢轨打磨量,建立综合优化目标函数,采用遗传算法并联合车辆轨道动力学仿真模型求解优化钢轨打磨廓形;最后,运用所建立的钢轨廓形优化设计模型计算分析不同优化策略的设计结果. 研究结果表明:同时考虑轮轨磨耗、轮轨接触应力和钢轨打磨量,优化后曲线外、内轨廓形平均磨耗指数相比初始廓形下降68.9%,内轨接触应力下降39.1%,打磨量下降21.8%,优化效果最佳;只考虑轮轨磨耗和接触应力时,优化后曲线外轨廓形磨耗指数和内轨接触应力下降较为明显,但打磨量下降速率相对较慢,仅为11.3%;只考虑打磨量时,优化后钢轨廓形打磨量下降最快,为24.4%,但轮轨接触应力显著变大.
Abstract:In order to minimize grinding amount in optimization design of heavy-haul rail grinding profile, an alignment and calculation method of the grinding amount of rail profile was established. Meanwhile, a comprehensive optimization evaluation model was designed. The model regarded wheel-rail wear index, wheel-rail contact stress and rail grinding amount as optimization sub-objectives, and the optimization results of different optimized strategies were analyzed. Firstly, an automatic alignment algorithm for rail profile was established through the theories of matrix rotation transformation, curve fitting and spline interpolation. Then the amount of rail grinding was calculated. Secondly, considering the optimization indicators such as wheel-rail wear index, contact stress and rail grinding amount, a comprehensive optimization objective function was established. The genetic algorithm was used to solve the optimized rail profile in conjunction with the vehicle track dynamics simulation model. Finally, the design results of different optimization strategies were calculated and analyzed by using the established rail profile optimization design model. The results show that, considering wheel-rail wear, wheel-rail contact stress and amount of rail grinding at the same time, the average wear index of the optimized high and low rail profile reduces by 68.9% when compared with the initial profile. The low rail contact stress obtains a decrease of 39.1%. The grinding amount gets a reduction of 21.8%. Thus the optimized effect is the best. After optimization, the high rail profile wear index and low rail contact stress decrease significantly in conditions of only considering wheel-rail wear and contact stress, but the decline rate of the grinding amount is relatively slow, reaching 11.3%. When only considering the grinding amount, the grinding amount of rail profile drops the fastest after optimization, which is 24.4%, while the wheel-rail contact stress is significantly larger.
-
重载线路因具有货运频繁、线路复杂、牵引重量大等特点,容易发生严重的轮轨磨耗损伤,尤其在重载曲线钢轨段,其损伤形式主要表现为钢轨侧磨和钢轨压溃[1],造成轮轨表面应力分布不良,影响轮轨动力学性能和列车运行安全. 轮轨接触几何型面的不平顺会造成轮轨表面接触应力的分布差异[2]及车辆结构疲劳等问题[3],进而引起钢轨损伤. 采用钢轨打磨目标设计廓形的方式,可有效改善轮轨几何型面匹配性能,预防钢轨接触疲劳,减轻钢轨损伤[4].
在钢轨廓形目标型面优化设计方面:Persson等[5]以列车脱轨系数、轮轨滚动接触疲劳、最大轮对横向位移等动力学指标作为惩罚因子加权建立罚函数,采用遗传算法得到优化性能较好的目标钢轨廓形;Choi等[6]以最小化曲线段钢轨磨损为优化目标,提出一种基于遗传算法的非对称式钢轨廓形设计方法,采用动力学理论分析评价轮轨力大小以及脱轨情况,所优化后得到的内外钢轨廓形相比初始廓形具有更小的磨耗指数;Wang等[7]引入轮轨接触几何关系建立廓形优化函数,运用Sequential Quadratic Programming算法求解得到匹配LM (标准磨耗型)车轮性能更好地打磨钢轨廓形;毛鑫等[8-9]基于轮径差函数曲线,建立直线和曲线钢轨打磨目标廓形的优化模型,得到轮轨接触分布良好的目标廓形;王亮等[10]利用NURBS (non-uniform rational b-splines)曲线构建以轮轨接触性能和轮对曲线通过能力Kriging代理模型为目标函数的非对称打磨目标型面多目标优化模型,采用NSGA-II (non dominated sorting genetic algorithm-II)算法优化使轮对曲线通过能力改善.
目前对于钢轨打磨廓形优化设计,主要通过改善轮轨接触分布和减少钢轨磨损等方式,并未在设计过程中直接考虑目标廓形打磨量. 肖杰灵等[11-12]提出钢轨打磨廓形设计应考虑打磨量以及打磨量最小原则,但并未涉及打磨量最小化的具体设计方法. 本文建立目标廓形打磨量计算方法,并将打磨量与主导重载线路钢轨损伤的磨耗损伤、压溃损伤进行整合,发展重载钢轨打磨廓形多目标优化设计方法,设计钢轨打磨廓形.
1. 钢轨打磨量计算方法
综合考虑轮轨磨耗指数、轮轨接触应力和打磨量因素,进行钢轨打磨目标廓形优化设计. 磨耗指数和轮轨接触应力可通过Simpack动力学软件仿真计算. 此外,通过建立任意钢轨廓形与目标钢轨廓形的对齐算法计算目标廓形打磨面积,考虑到用钢轨横截面形状即可描述钢轨廓形几何特征,因此,用钢轨横截面面积变化量表征打磨量,并将打磨量整合到廓形优化模型中. 其中,目标廓形源于通过遗传优化算法选择、交叉、变异算子自动创建的钢轨廓形,优化迭代结束后最后一代廓形中综合性能最好的个体即为打磨廓形.
1.1 实测廓形分析
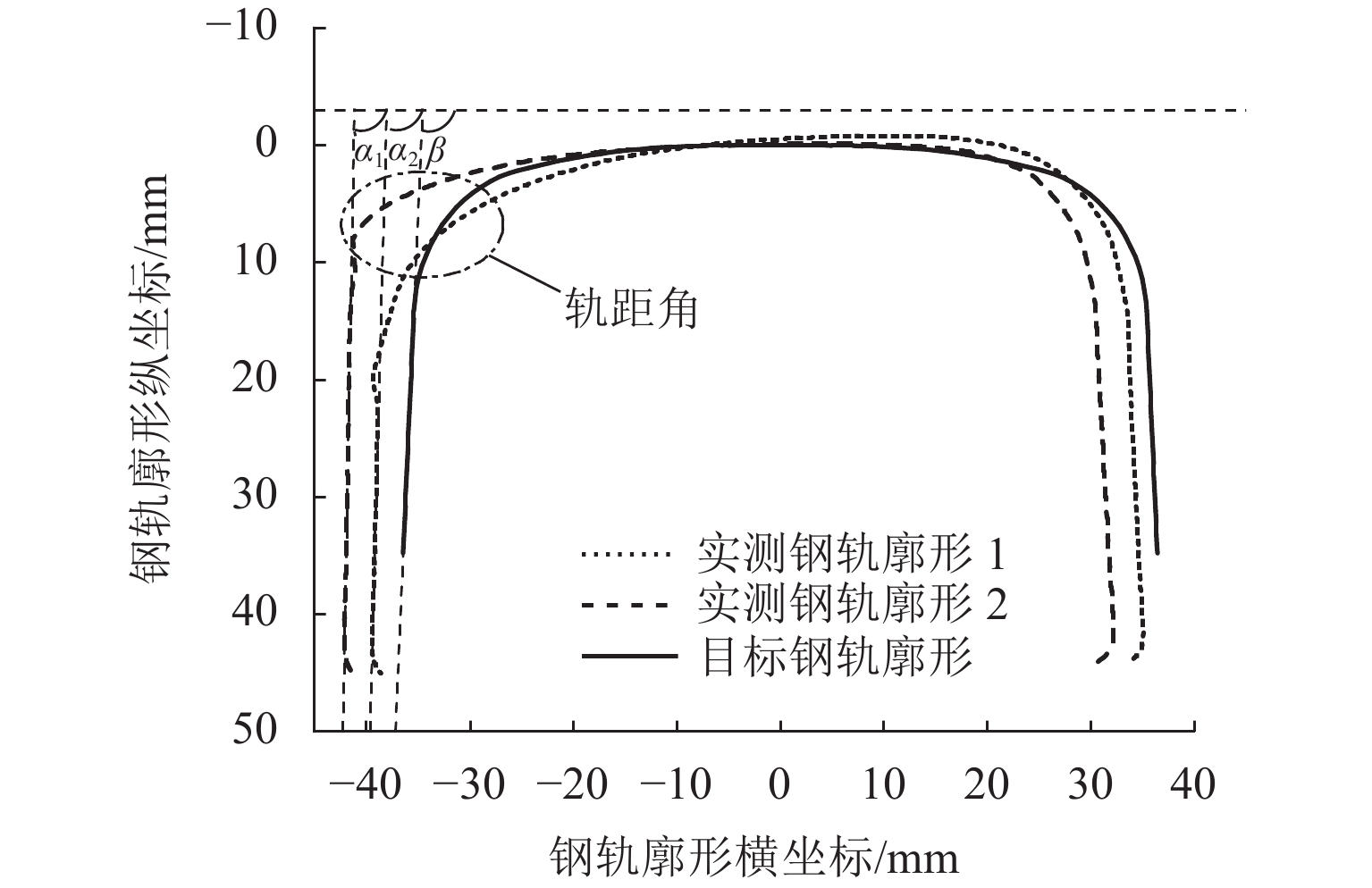
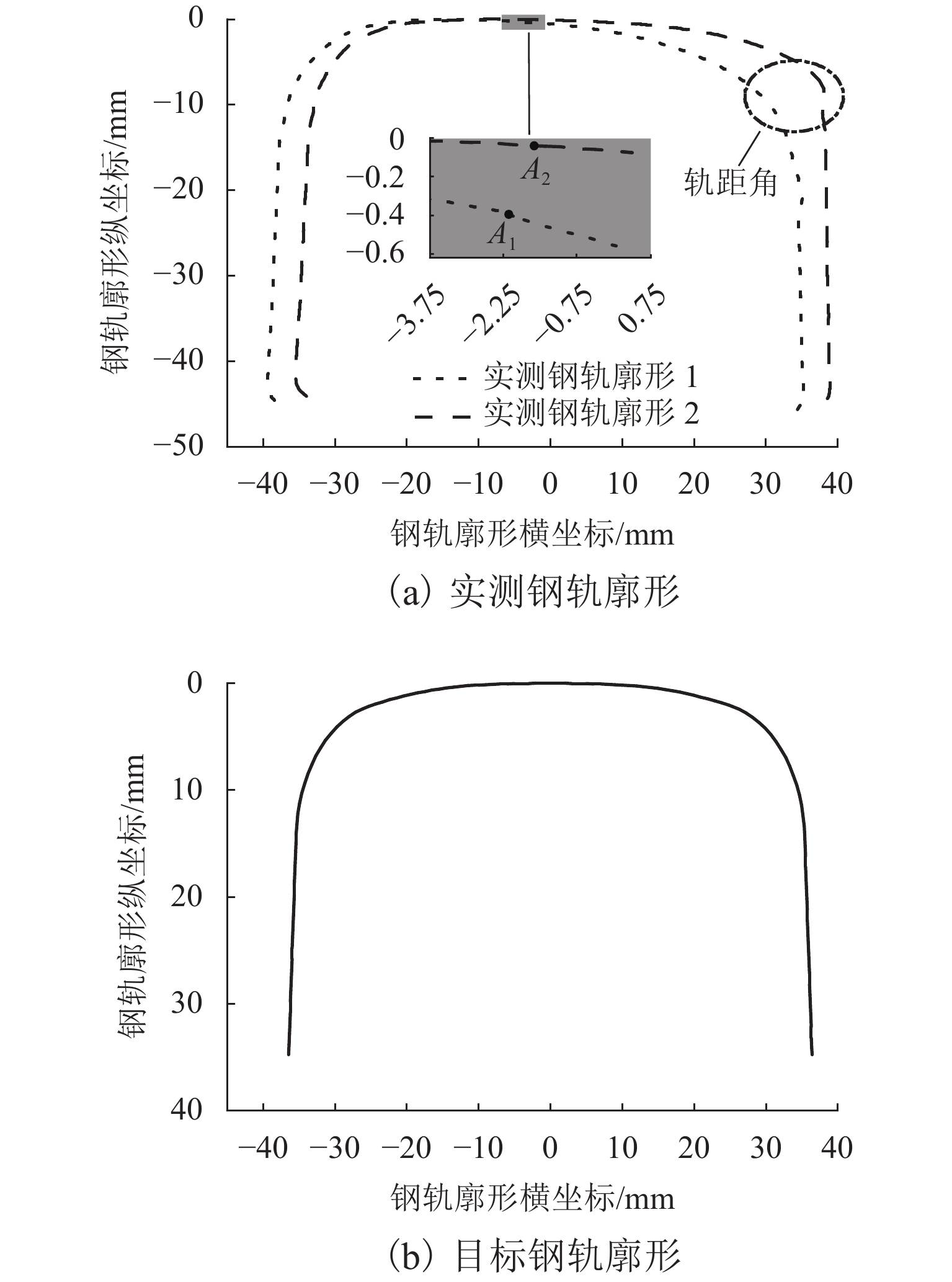
测得某线路不同里程段钢轨廓形,选取具有代表几何特征的两种实测钢轨型面进行分析. 如图1(a)所示,曲线段实测钢轨廓形1轨距角处的钢轨磨损相比较实测钢轨廓形2更为严重,A1、A2两点分别为实测廓形1和2轨顶处的两点. 此外,图1(b)打磨目标廓形与实测廓形坐标正方向相反,由于打磨目标廓形需要输入到车辆-轨道动力学模型中计算,而根据Simpack建立的车辆−轨道动力学模型系统以打磨目标廓形坐标正方向为标准. 为了计算实测廓形打磨成目标廓形之后的材料去除截面面积,需以目标廓形坐标朝向为基准对实测钢轨廓形坐标进行旋转变换预处理,使打磨目标钢轨廓形与实测廓形进行重叠放置.
图1(a)中,将A1、A2作为旋转变换中心,通过编写数值程序自动找到该旋转中心点,根据式(1)对两种实测廓形分别绕A1、A2进行旋转变换预处理,使其靠近预期目标廓形,如图2所示. 图2中:α1、α2、β为通过反三角函数arctan k1、arctan k2、arctan k0求得的夹角.
Rph=RinihMsphMrotMsmh,h=1,2, (1) 式中:Rph为实测廓形h旋转变换处理后的矩阵; Msph为初始实测廓形h的负向平移矩阵,如式(2);Mrot为绕原点旋转的旋转矩阵,如式(3);Msmh为初始实测廓形h的正向平移矩阵,如式(4);Rinih为实测廓形h初始矩阵,如式(5).
Msph=[10−xh01−yh001], (2) Mrot=[cosθ−sinθ0sinθcosθ0001], (3) Msmh=[10xh01yh001], (4) Rinih=[RxhRyhE]n×3, (5) 式中:(xh,yh)为旋转中心点横、纵坐标;θ为绕原点的旋转角度,此处值为π;Rxh、Ryh分别为初始实测廓形h数据点的横、纵坐标向量;E为单位列向量.
1.2 钢轨廓形横向对齐
图2中两实测钢轨廓形通过旋转变换已被置于预期目标廓形附近,为了使实测廓形与目标廓形轨距角侧斜直线段重合,需要再次旋转实测廓形,使其与目标廓形内侧斜直线在误差允许范围内斜率相等. 旋转角度计算方法如下所述.
1) 保持目标廓形不动,绕坐标原点旋转上述预处理后的实测廓形,使其内侧与预期目标钢轨廓形平行. 根据图2可知斜率及旋转角度为
ki=n∑jn=1yjn(xjn−ˉx)n∑jn=1(xjn−ˉx)2,i=0,1,2, (6) δi=arctanki−arctank0, (7) 式中:k1、k2、k0均为实测廓形1、2以及目标对齐廓形内侧斜直线斜率,实际中廓形轨距角侧(即钢轨内侧)廓形段经常为近似直线的曲线,可通过最小二乘法对钢轨廓形内侧曲线段进行线性拟合得到斜直线及其斜率;δi为旋转角度;jn为选取廓形内侧近似斜直线段的离散点编号;n为该斜直线段离散点数目;xjn、yjn分别为该段点的横、纵坐标;
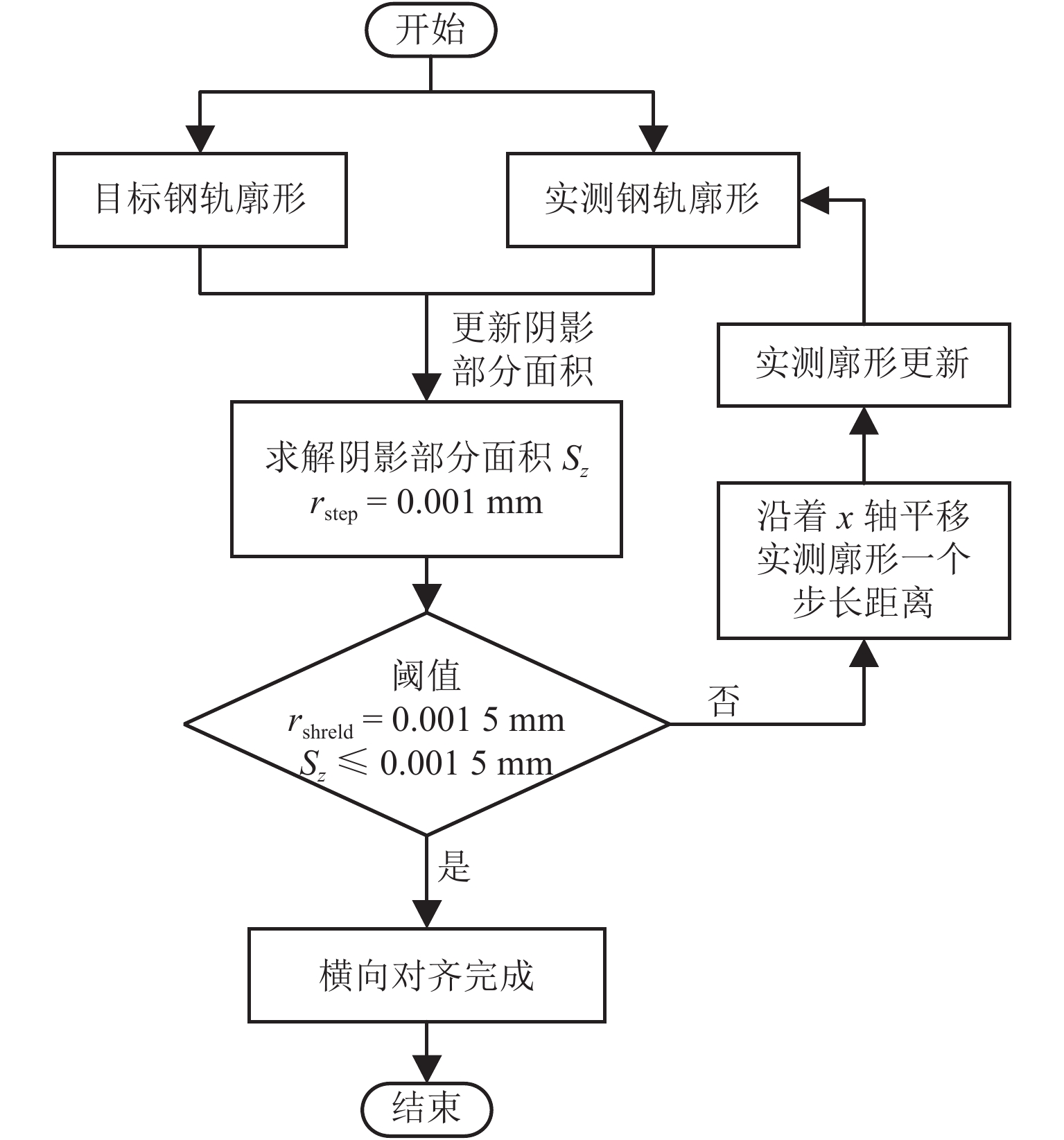
¯x 为该段数据横坐标平均值.根据式(1)计算并获得与目标廓形内侧斜直线段平行的实测廓形矩阵,但在该公式中,初始矩阵为Rph,旋转中心为原点(0,0),旋转矩阵Mrot中θ值为δ,其结果如图3所示,阴影部分面积Sz为两廓形内侧段某区间[13]直线围成的面积,z为大于等于1的正整数.
2) 对上述处理后的实测钢轨廓形左、右平移,构建平移向量,使其与目标廓形内侧斜直线段重合,其迭代流程见图4. 采用最小面积法判断内侧斜直线是否重合[14],如式(8).
Sz⩽ε, (8) 式中:ε为精确度,即面积阈值(rshreld),取为0.0015 mm.
选取纵坐标区间长度为5 mm,设置移动步长为0.001 mm,则可得到实测廓形的单次平移矩阵为
R1=[r step 0], (9) 式中:rstep为移动步长,表示沿x轴方向移动rstep距离,0表示沿y轴方向不进行平移.
通过不断迭代移动步长改变阴影部分面积,使该面积满足式(8)时,即可判定钢轨横向对齐完成,对齐效果见图5.
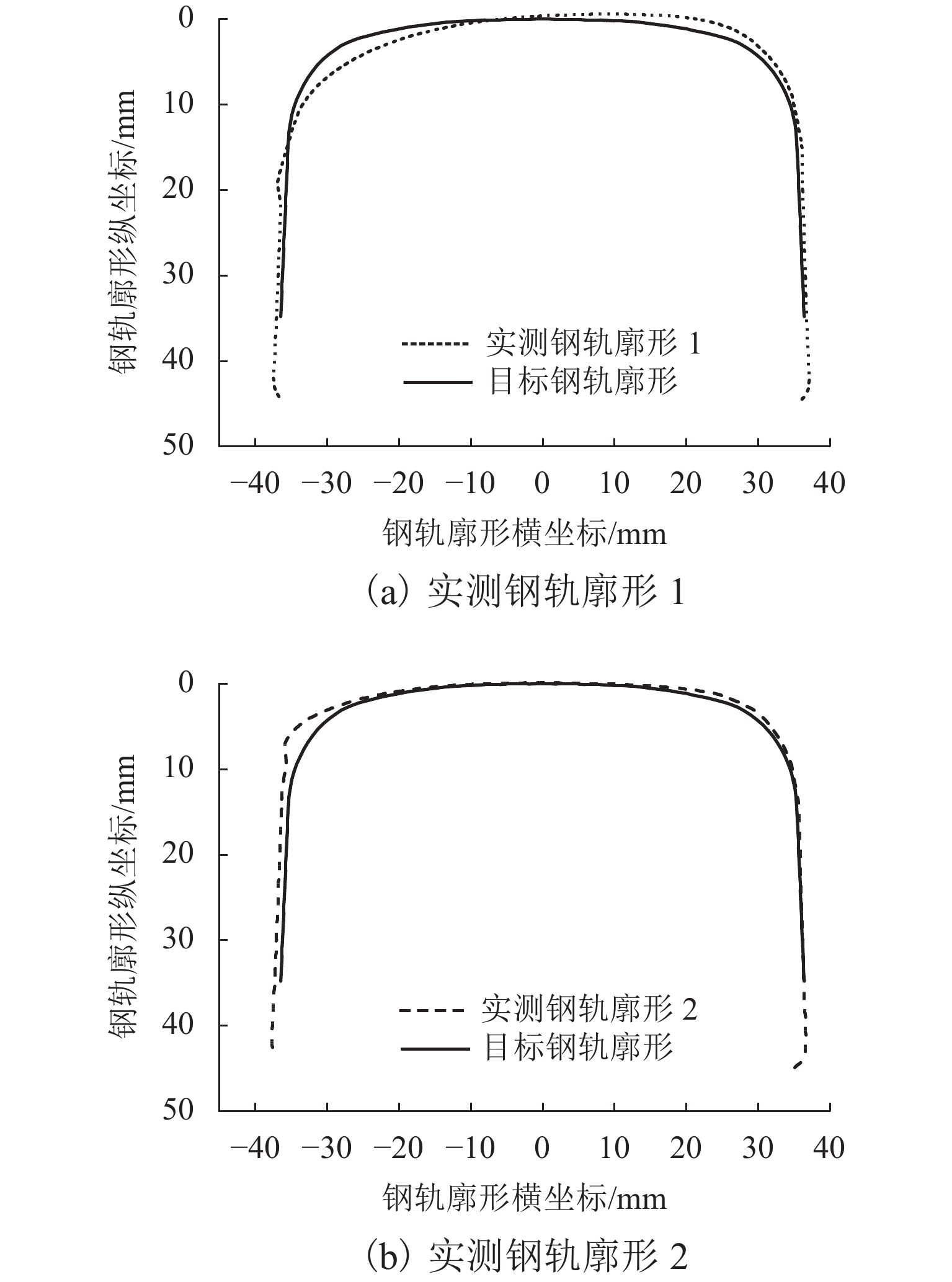
由图5可知:在轨距角处,相比较实测廓形2,目标廓形并未被实测廓形1所包裹,实测廓形1并不能打磨成目标廓形的样子.
1.3 钢轨廓形垂向对齐
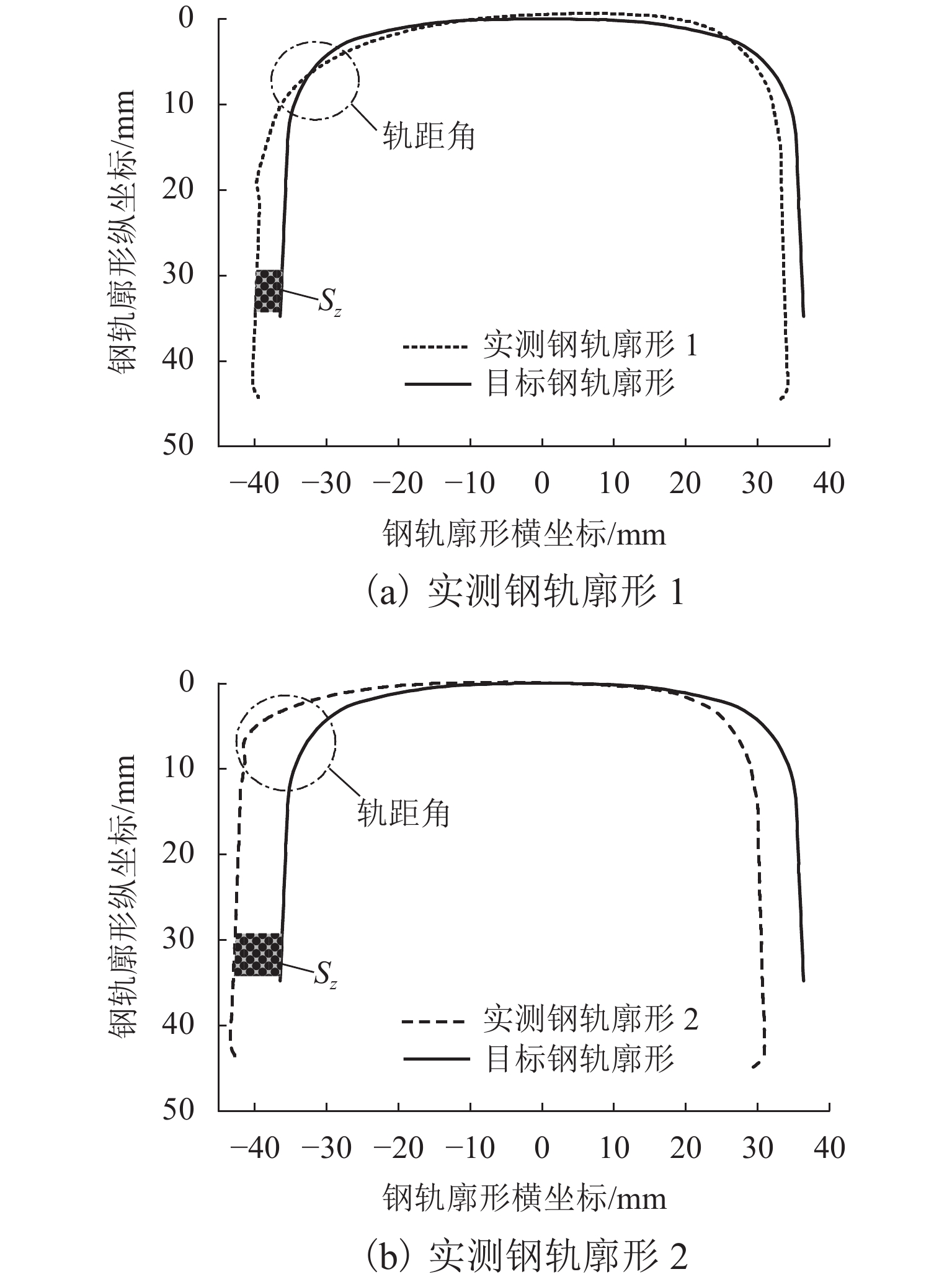
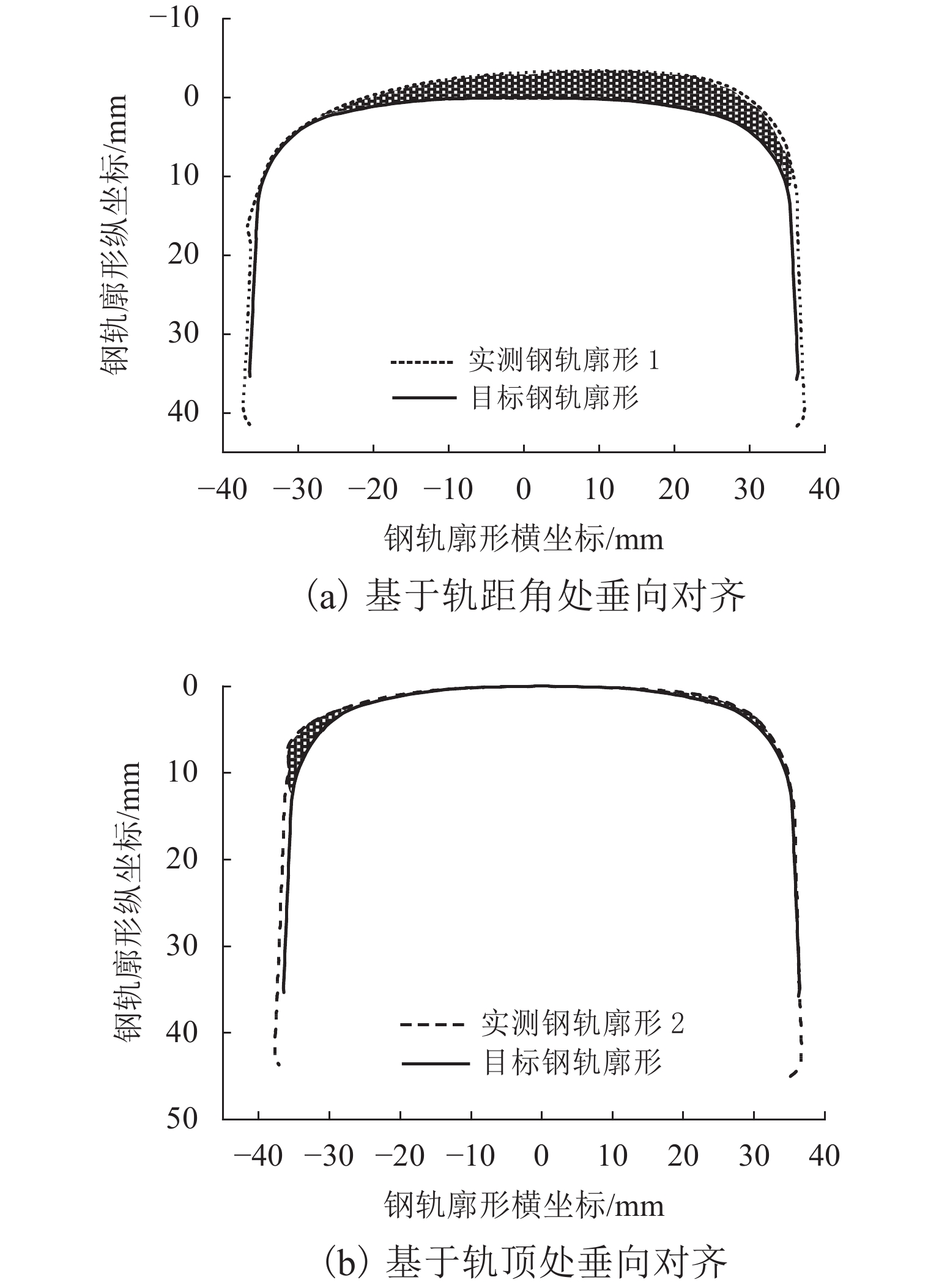
在做垂向对齐算法设计时,需根据不同实测廓形轨距角处磨损差异,基于轨距角和轨顶建立不同的垂向对齐方式. 基于轨距角处对实测钢轨廓形1垂向平移,使其在轨距角处对齐,结果如图6(a)所示. 基于轨顶最高处对实测钢轨廓形2垂向平移,使其在轨顶处对齐,结果如图6(b)所示.
1.4 实测钢轨廓形打磨量计算方法
图6(a)和6(b)中的阴影部分均为由钢轨廓形曲线围成的不规则形状,计算出该面积即可知所要求解的钢轨总打磨量. 由于实际钢轨廓形数据是二维离散坐标点,且相邻离散点间距离足够小,可将图中阴影部分看作封闭的多边形,即将两廓形曲线的横纵坐标分别拼接为组成该多边形的两个向量,见式(10),采用求任意多边形面积的计算方法即可求出该钢轨廓形打磨面积的近似值,该近似值满足本文优化所需打磨量参数条件.
{X=[x1x2⋯xc⋯xnt−1x1]T,Y=[y1y2⋯yc⋯ynt−1y1]T,Sg = 0.5|nt∑m=1(xmym+1−xm+1ym)|, (10) 式中:X、Y为实测廓形和目标廓形阴影部分横、纵坐标组成的向量,其中,前c个点为实测钢轨廓形1或2的坐标点,后nt−1−c个点为目标钢轨廓形坐标点,最后再添加点(x1, y1)是为了让阴影面积对应两曲线构成封闭多边形;Sg为钢轨打磨面积;[xm ym]、[xm + 1 ym + 1]为X、Y第m和m + 1个点组成的向量;nt为围成打磨面积区域(即图6中阴影部分)的廓形点数目.
2. 打磨廓形优化方法
轮轨磨耗和轮轨接触应力是描述轮轨相互作用的两个重要指标,将钢轨打磨为特定的几何形状可以显著改善磨耗和应力. 此外,钢轨打磨量过大会缩减钢轨服役寿命并消耗过多的打磨资源. 因此,本文采用轮轨磨耗指数、轮轨接触应力和钢轨打磨量为设计钢轨打磨廓形的子目标函数,通过加权法整合,构建综合目标函数. 用Simpack建立C80货车车辆−轨道动力学模型,联合MATLAB数值计算软件编写遗传数值优化算法求解目标函数最优解,得到钢轨打磨目标廓形. 需要说明的是第1节中的目标廓形是为了介绍廓形对齐及打磨量计算方法而引出,具有任意代表性,而此处的打磨目标廓形是通过廓形优化设计方法输入特定的工况参数得到的目标廓形.
2.1 综合优化目标函数
根据文献[15]设计钢轨廓形变量C,分别赋予轮轨磨耗指数、轮轨接触应力和钢轨打磨量不同的影响权重系数并加权建立综合优化目标函数,如式(11),其中,轮轨磨耗指数和接触应力均选择车辆稳定运行部分的平均磨耗指数和轮轨接触应力作为评判优化廓形性能的磨耗和应力指标.
F(C)=3∑iid=1wiidfiid(C), (11) 式中:F(C)为综合优化目标函数值;
w1id 为轮轨磨耗指数权重,w2id 为轮轨接触应力权重,w3id 为打磨量权重;f1id (C)为轮轨磨耗指数归一化指标,f2id (C)为轮轨接触应力归一化指标[16],f3id (C)为上述计算的打磨量Sg归一化指标.2.2 目标函数最优解
获得最优钢轨打磨廓形个体就是求取综合优化目标函数的最优解,即目标函数的最小值. 遗传算法因具有良好的全局适应性和全局搜索性经常被用于求解优化问题.
选取实际重载线路段不同的实测钢轨廓形作为初始种群,这些廓形分别来自直线位置和曲线位置,它们新铺设时均为标准CN60轨廓形、轨底坡1∶40. 运用改进的NURBS (non-uniform rational b-splines)曲线构造方法[17]对钢轨廓形进行参数化表示,将其导入建立的Simpack车辆动力学模型中,计算得到轮轨的磨耗指数、轮轨接触应力. 运用第1节提到的钢轨打磨量计算方法计算出实测廓形打磨成任意目标种群廓形的打磨量,并进行数值归一化计算,得出每代种群不同廓形个体的目标函数值F(C),挑选每代最优个体(目标函数最小值对应个体)进行后续计算结果分析,取其倒数为算法的适应度值,根据此值筛选出符合设计要求的廓形个体作为父代个体进行选择、交叉、变异等操作产生下一子代廓形个体,经过多次迭代更新,达到算法最大迭代次数后,目标廓形更新结束,选取最后的子代目标函数值最小的个体作为最优设计的钢轨打磨廓形.
2.3 轮轨磨耗指数
轮轨磨耗指数为
W=|Txξx|+|Tyξy|+|Tϕξϕ|, (12) 式中:Tx、Ty、T
ϕ 分别为接触点的纵向蠕滑力、横向蠕滑力和自旋蠕滑力矩;ξx 、ξy 、ξϕ 分别为轮轨接触斑处的纵向蠕滑率、横向蠕滑率和自旋蠕滑率.3. 优化方法的应用
选择第1节中提到的实测钢轨廓形1,应用第2节钢轨打磨廓形优化方法,选取不同的权重参数建立不同的打磨廓形设计优化策略分析轮轨磨耗指数、轮轨接触应力、钢轨打磨量的迭代演化情况.
3.1 优化策略
以重载C80车动力学参数模型为载荷工况,并保持工况载荷条件不变,对不同优化子目标赋予不同的影响权重系数,分析不同权重系数的优化策略对优化结果的影响,具体参数见表1.
表 1 优化策略Table 1. Optimization strategies工况 磨耗权重系数 应力权重系数 打磨量权重系数 1 0.0833 0.1095 0.8072 2 0.4585 0.5515 0 3 0 0 1 工况1同时考虑了轮轨磨耗、接触应力以及钢轨打磨量的子目标,主要对轮轨磨耗指数、轮轨接触应力和钢轨打磨量均进行重点优化;工况2同时考虑对磨耗指数和接触应力子目标进行优化;工况3对磨耗指数和接触应力权重均赋予0,只考虑打磨量因素,主要针对打磨量子目标进行优化.
3.2 钢轨廓形迭代演化分析
动力学分析结果表明,钢轨廓形迭代计算的变化对车辆4个轮对均具有相同的影响趋势,尤其一位轮对,即车辆运行的导向轮对,变化速率更为明显. 每代遗传保留下来的钢轨廓形几何型面有多种,选取一位导向轮对计算该轮对分别和该多种钢轨廓形轮轨磨耗指数及接触应力的平均值,同时计算钢轨打磨量的最大值,分析每代钢轨廓形各优化目标的迭代演化情况.
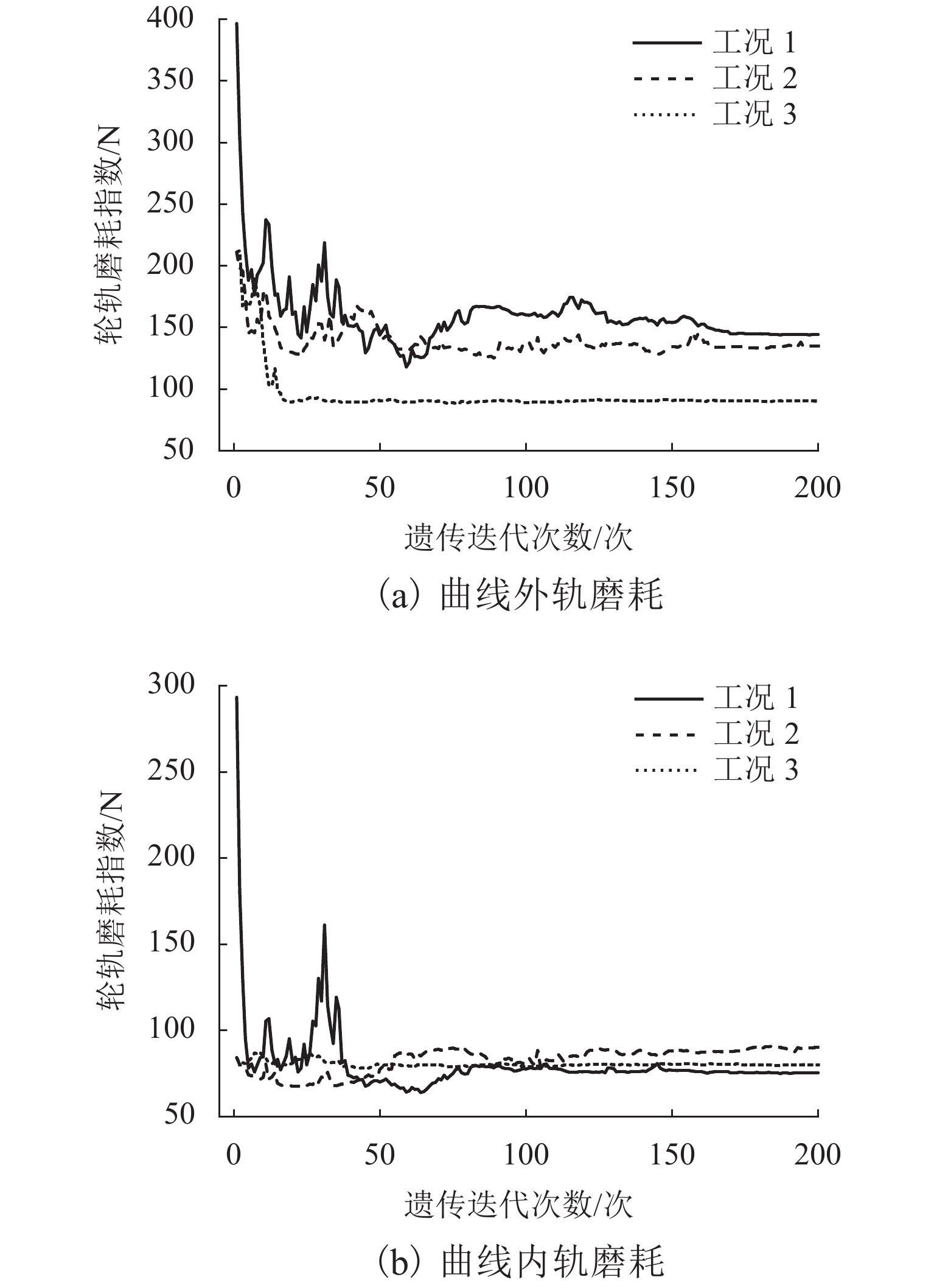
1) 轮轨磨耗指数迭代演化
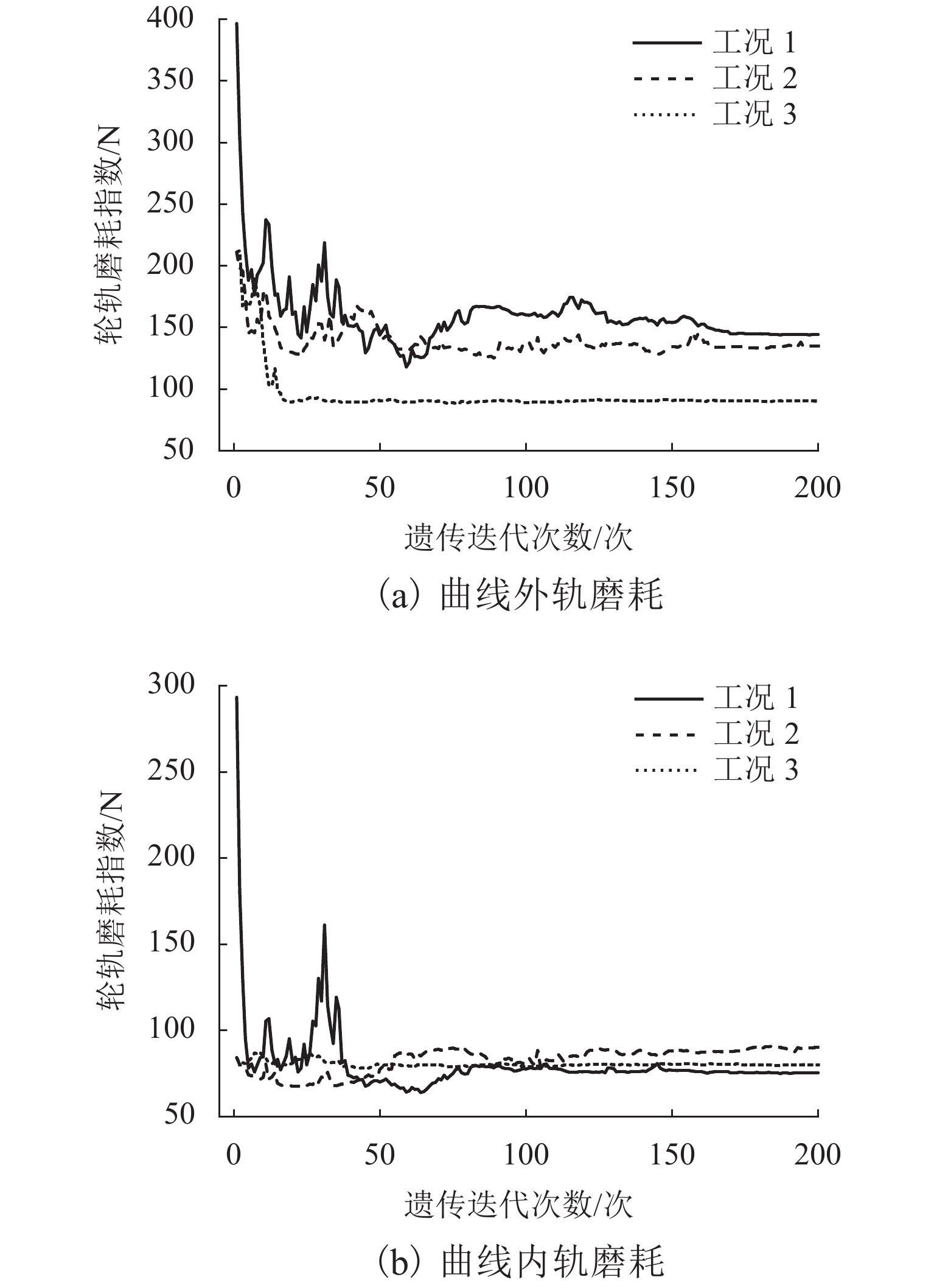
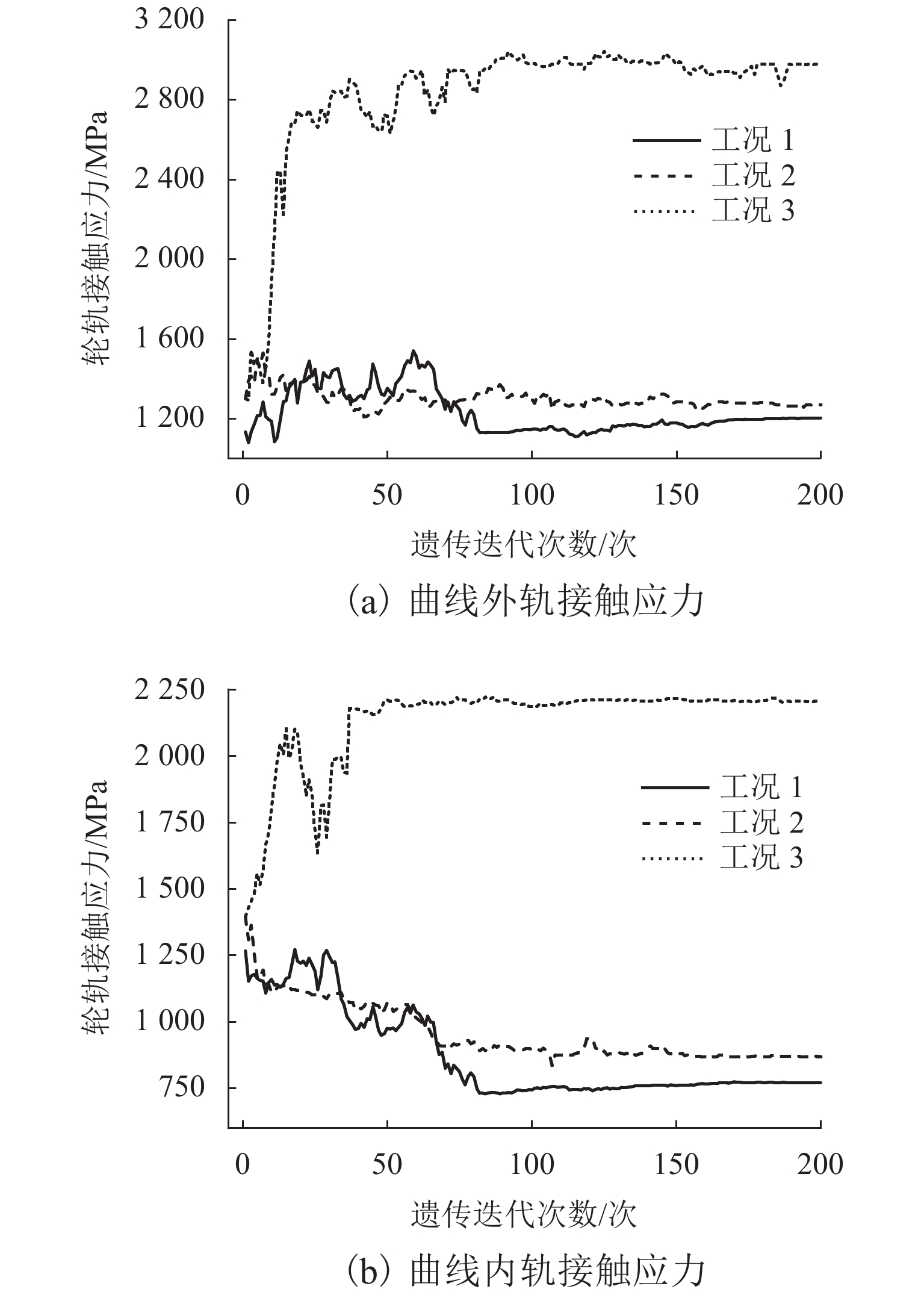
图7为一位轮对曲线外轨和内轨的轮轨磨耗指数迭代演化过程,由图7可知:工况1的轮轨磨耗指数变化速率最大,收敛时相比初始廓形外轨和内轨平均降低了68.9%;3种工况对于外轨的磨耗指数优化效果更明显,均呈下降趋势,而工况2、3对于内轨的磨耗指数优化前后无明显变化;同时考虑磨耗、应力和打磨量的优化策略对轮轨磨耗优化效果最好.
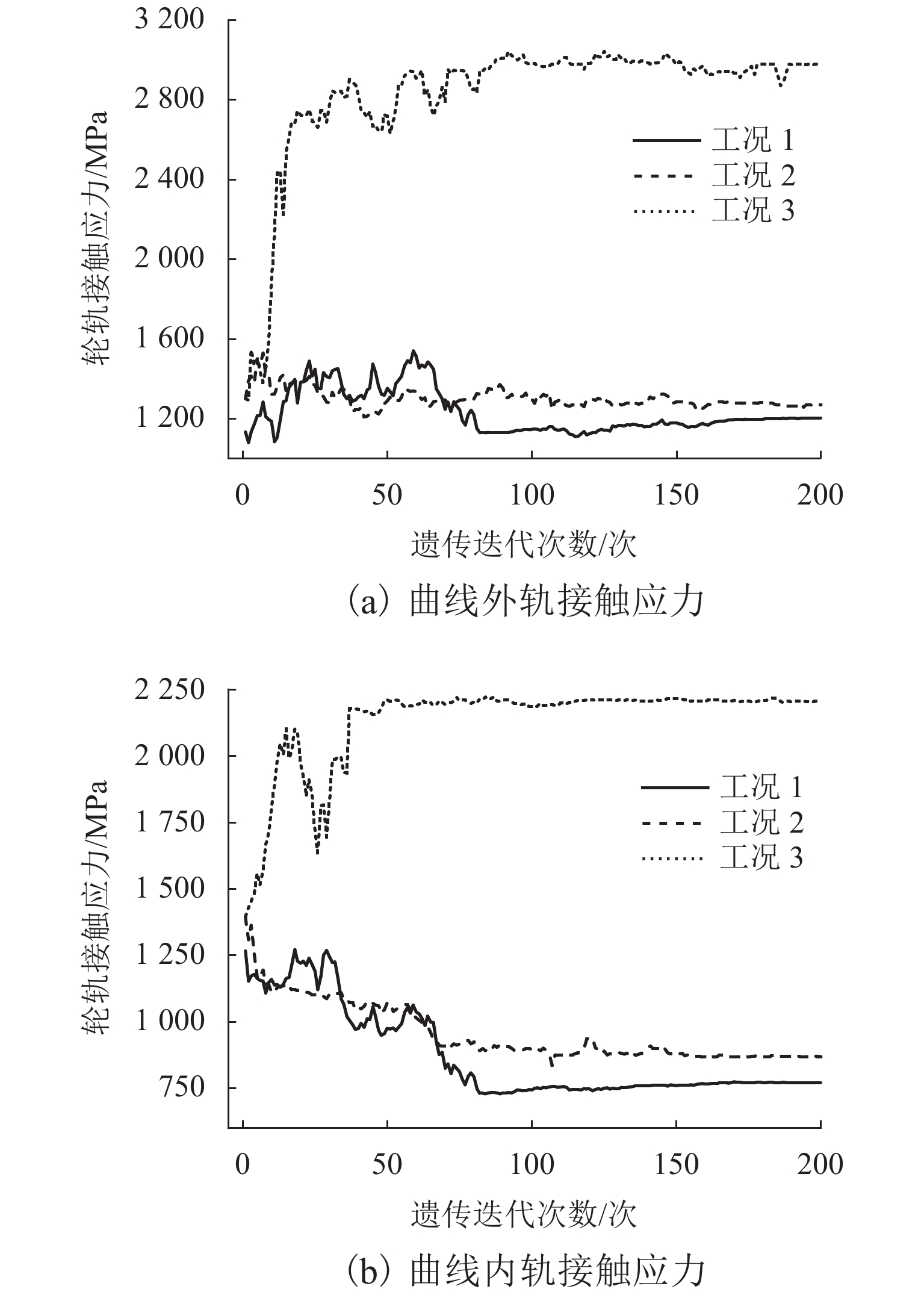
2) 轮轨接触应力的迭代演化
图8为一位轮对接触应力指数迭代演化过程,分析图8可知:工况1、2对于内轨的接触应力优化效果更为明显;工况1下降速率最快,收敛状态下比初始廓形内轨接触应力下降了39.1%,而工况2下降了37.7%;工况3对于轮轨接触应力指标起到了负优化的作用,其外轨和内轨接触应力值相比初始廓形增长幅度明显,表明该工况下得到的优化钢轨廓形与该车辆模型中的车轮匹配,其轮轨接触应力过大,而较大的轮轨接触应力会加速钢轨接触疲劳,应尽力避免.
此外,图8工况3接触应力最大的原因是轮对通过曲线时,曲线外轨更容易在轨肩处接触,轨肩处钢轨廓形曲率半径小,使得接触应力大;图7中工况3磨耗指数小的原因是曲线外轨轨肩接触,增加了轮径差,轮对通过曲线时曲线外轨的轮轨蠕滑率下降,进而导致磨耗指数下降. 综上所述,同时考虑磨耗和应力的工况2对轮轨接触应力优化效果最好,而只考虑打磨量的工况3优化效果最差.
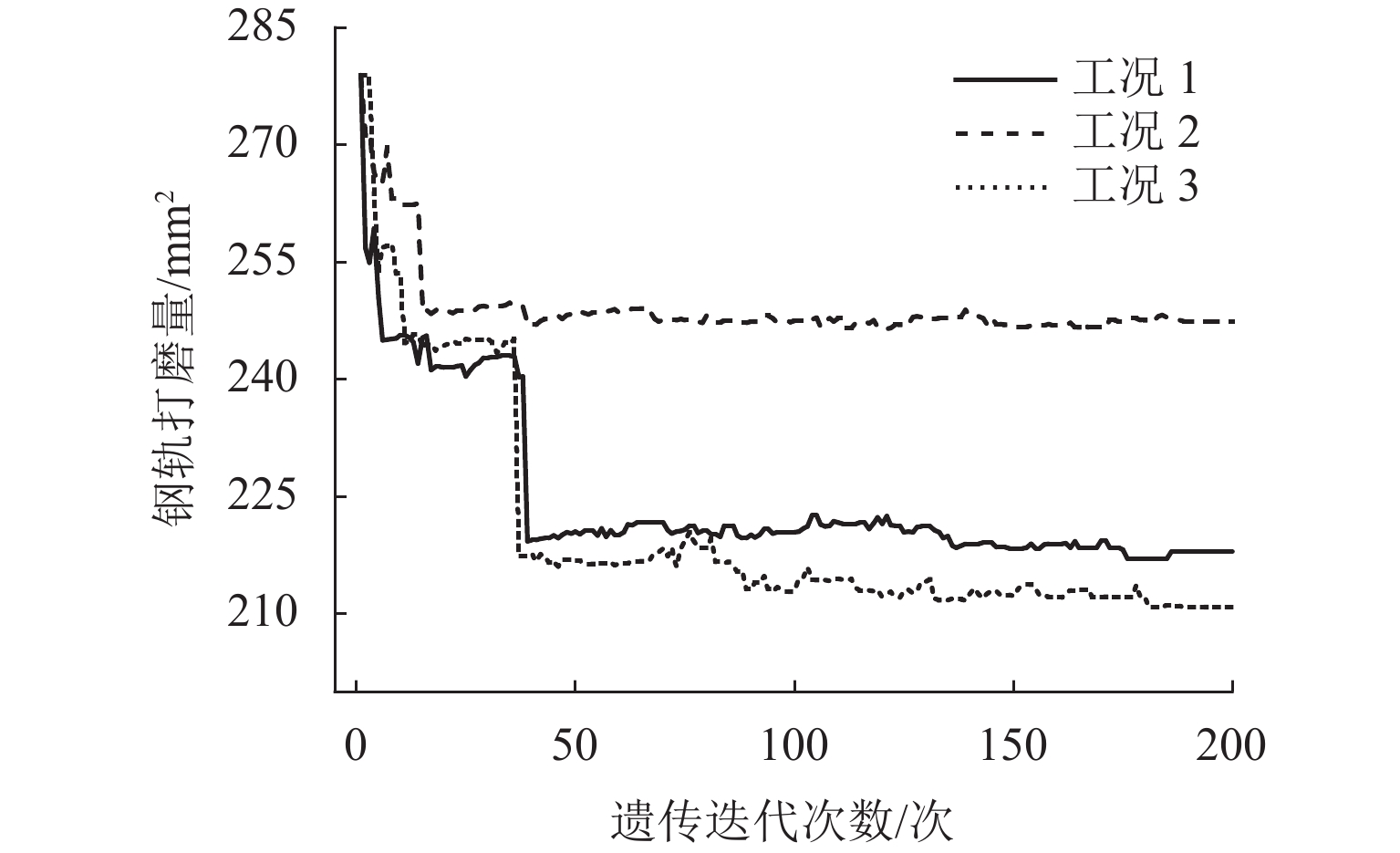
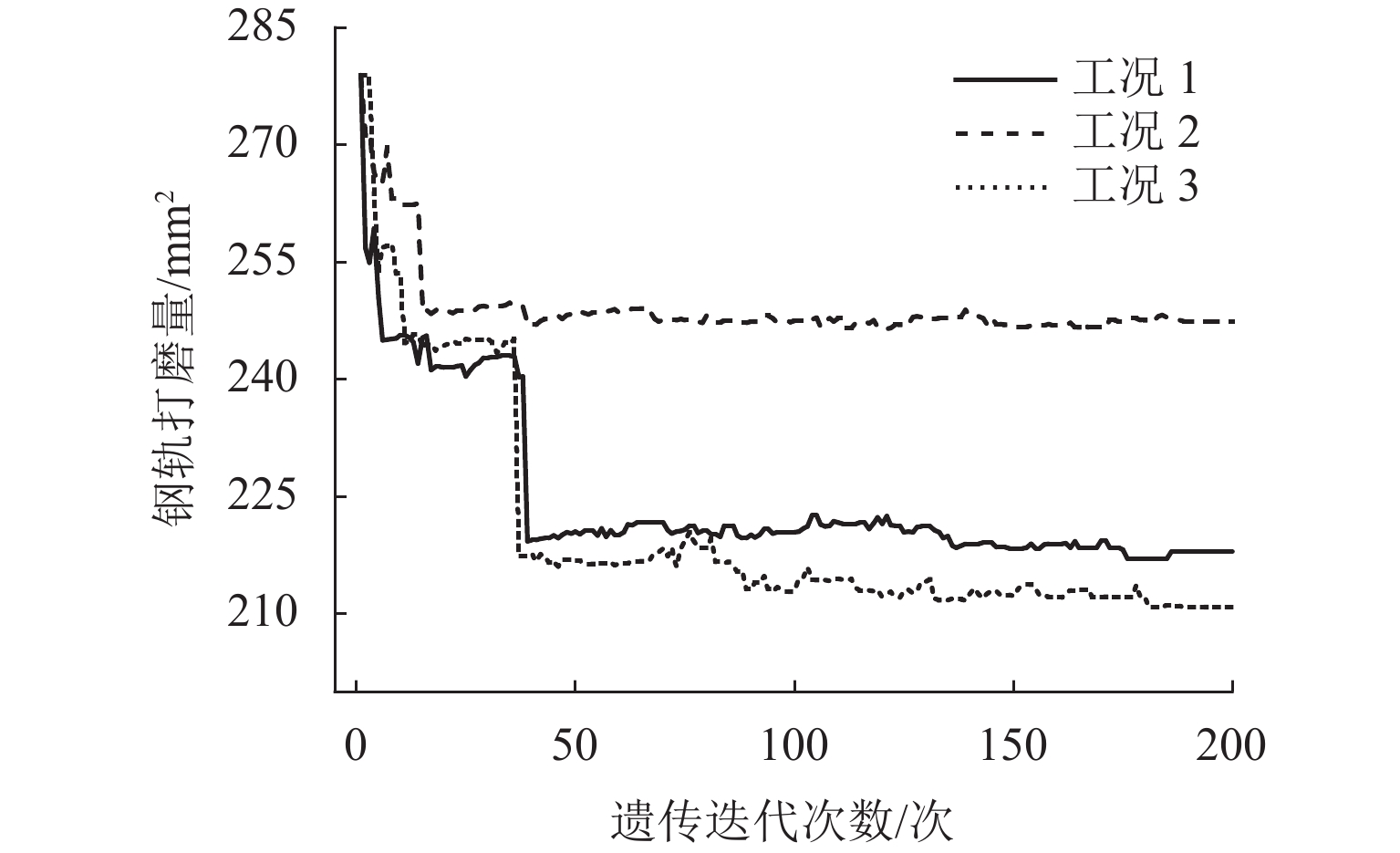
3) 钢轨打磨量的迭代演化
图9为钢轨打磨量指数迭代演化过程,由图9可知:工况1、2、3的每代钢轨廓形最大打磨量下降均很明显,并都在37代附近收敛;第200代廓形相比初始廓形分别降低了21.8%、11.3%、24.4%,工况3变化速率最大.
综上可知:工况1由于给三者均分配了权重系数,因而对于曲线外、内轨的轮轨磨耗指数,内轨的接触应力以及钢轨的打磨量均具有较好的优化效果;工况2对于外轨磨耗指数,内轨接触应力优化效果明显,但由于没考虑打磨量因素,打磨量下降速率相比较其他两种工况较慢,优化效果不够明显;工况3由于打磨量权值为1,占权值全部比重,打磨量下降速率最大,根据打磨量最小化原则,打磨量指标优化效果最好,但曲线钢轨接触应力却显著增大,可能加大轮轨损伤,应当避免. 因此,综合3种指标迭代演化过程可知,同时考虑磨耗、应力和打磨量的工况1对钢轨廓形优化效果最好.
3.3 最优廓形性能分析
考虑到重载曲线钢轨损伤以曲线外轨磨耗、内轨压溃为主的特点,用外轨磨耗指数和内轨接触应力来评价设计廓形好坏,作为确定钢轨打磨廓形设计方案的依据. 选取不同工况最优廓形,得轮轨磨耗指数和接触应力如表2所示.
表 2 外轨磨耗和内轨接触应力Table 2. High rail wear and low rail contact stress工况 外轨磨耗指数/N 内轨应力/MPa 实测廓形 1 2482.0 1294.0 1 105.1 770.5 2 133.8 868.3 3 109.8 2201.0 分析表2可知:与实测廓形1相比,工况1最优廓形外轨磨耗及内轨接触应力下降最显著;工况3对于轮轨磨耗优化效果明显,但却导致接触应力急剧上升,因此对于应力呈负优化效果. 综上所述,同时考虑磨耗、应力和打磨量的工况1效果最好.
4. 结 论
1) 建立了基于目标廓形的钢轨打磨廓形自动对齐算法和打磨量计算方法,该方法可以计算任意测试廓形与目标廓形的打磨量. 将打磨量整合到钢轨打磨廓形优化设计综合评价函数中,发现优化迭代可以显著降低打磨量.
2) 所建立的钢轨打磨廓形优化设计模型可以差异化地最小化轮轨磨耗、轮轨接触应力以及钢轨目标廓形打磨量. 在优化过程中只考虑打磨量因素时,打磨量和轮轨磨耗会降低,但轮轨接触应力会显著增加.
3) 同时考虑轮轨磨耗、轮轨接触应力、打磨量的影响时,或只考虑磨耗和应力的影响时,磨耗、应力显著下降,但前者所得目标廓形所需的打磨量更少. 因此,在钢轨打磨目标廓形优化设计中,有必要同时考虑磨耗、应力和打磨量的影响.
-
表 1 优化策略
Table 1. Optimization strategies
工况 磨耗权重系数 应力权重系数 打磨量权重系数 1 0.0833 0.1095 0.8072 2 0.4585 0.5515 0 3 0 0 1 表 2 外轨磨耗和内轨接触应力
Table 2. High rail wear and low rail contact stress
工况 外轨磨耗指数/N 内轨应力/MPa 实测廓形 1 2482.0 1294.0 1 105.1 770.5 2 133.8 868.3 3 109.8 2201.0 -
[1] 刘启跃,王文健,周仲荣. 高速与重载铁路钢轨损伤及预防技术差异研究[J]. 润滑与密封,2007,32(11): 11-14,68. doi: 10.3969/j.issn.0254-0150.2007.11.004LIU Qiyue, WANG Wenjian, ZHOU Zhongrong. An investigation on difference of rail damage and preventive technique of high-speed and heavy-haul railway[J]. Lubrication Engineering, 2007, 32(11): 11-14,68. doi: 10.3969/j.issn.0254-0150.2007.11.004 [2] GERLICI J, LACK T. Contact geometry influence on the rail/wheel surface stress distribution[J]. Procedia Engineering, 2010, 2(1): 2249-2257. [3] 董勇,康彦兵,张华鹏,等. 地铁线路钢轨波磨对车辆振动特性的影响[J]. 机械,2021,48(10): 22-29. doi: 10.3969/j.issn.1006-0316.2021.10.004DONG Yong, KANG Yanbing, ZHANG Huapeng, et al. Effect of rail corrugation in metro line on vibration characteristics of vehicle[J]. Machinery, 2021, 48(10): 22-29. doi: 10.3969/j.issn.1006-0316.2021.10.004 [4] GRASSIE S, NILSSON P, BJURSTROM K, et al. Alleviation of rolling contact fatigue on Sweden's heavy haul railway[J]. Wear, 2002, 253(1/2): 42-53. [5] PERSSON I, NILSSON R, BIK U, et al. Use of a genetic algorithm to improve the rail profile on Stockholm underground[J]. Vehicle System Dynamics, 2010, 48(S1): 89-104. [6] CHOI H Y, LEE D H, SONG C Y, et al. Optimization of rail profile to reduce wear on curved track[J]. International Journal of Precision Engineering and Manufacturing, 2013, 14(4): 619-625. doi: 10.1007/s12541-013-0083-1 [7] WANG P, GAO L, XIN T, et al. Study on the numerical optimization of rail profiles for heavy haul railways[J]. Proceedings of the Institution of Mechanical Engineers, Part F:Journal of Rail and Rapid Transit, 2017, 231(6): 649-665. [8] 毛鑫,沈钢. 基于轮径差函数的曲线钢轨打磨廓形设计[J]. 同济大学学报(自然科学版),2018,46(2): 253-259. doi: 10.11908/j.issn.0253-374x.2018.02.017MAO Xin, SHEN Gang. Curved rail grinding profile design based on rolling radii difference function[J]. Journal of Tongji University (Natural Science), 2018, 46(2): 253-259. doi: 10.11908/j.issn.0253-374x.2018.02.017 [9] 郭战伟. 普速铁路钢轨打磨对轮轨接触关系的影响[J]. 中国铁道科学,2020,41(6): 109-116. doi: 10.3969/j.issn.1001-4632.2020.06.12GUO Zhanwei. Influence of rail grinding on wheel-rail contact relationship in general speed railway[J]. China Railway Science, 2020, 41(6): 109-116. doi: 10.3969/j.issn.1001-4632.2020.06.12 [10] 王亮,向伟彬,刘宏达,等. 小半径曲线钢轨非对称打磨目标型面优化[J]. 机械科学与技术,2021,40(6): 949-954.WANG Liang, XIANG Weibin, LIU Hongda, et al. Target profile optimization of asymmetrical grinding for rail with sharp-radius curve[J]. Mechanical Science and Technology for Aerospace Engineering, 2021, 40(6): 949-954. [11] 肖杰灵,刘学毅. 钢轨非对称廓型的设计方法[J]. 西南交通大学学报,2010,45(3): 361-365. doi: 10.3969/j.issn.0258-2724.2010.03.007XIAO Jieling, LIU Xueyi. Design method of rail asymmetric silhouette[J]. Journal of Southwest Jiaotong University, 2010, 45(3): 361-365. doi: 10.3969/j.issn.0258-2724.2010.03.007 [12] 张金,俞喆,杨超,等. 普速铁路钢轨打磨廓形优化设计及效果评价[J]. 铁道建筑,2020,60(10): 138-141.ZHANG Jin, YU Zhe, YANG Chao, et al. Optimization design and effect evaluation of rail grinding profile for conventional speed railway[J]. Railway Engineering, 2020, 60(10): 138-141. [13] 余博. 基于面积信息的钢轨打磨方法研究与实现[D]. 武汉: 武汉理工大学, 2018. [14] 黄佳乐,桂卫东. 基于MATLAB钢轨廓形法线值计算及现场应用[J]. 铁路技术创新,2020(2): 72-78. [15] 唐彦玲,吴磊,董勇,等. 重载铁路曲线钢轨廓形多目标优化设计[J]. 机械,2020,47(12): 1-9.TANG Yanling, WU Lei, DONG Yong, et al. Multi-objective optimization design of rail profiles on curve tracks of heavy haul railway[J]. Machinery, 2020, 47(12): 1-9. [16] 成棣,胡晓依,刘丰收,等. 基于改进层次分析法的高速列车轮轨型面匹配评价方法及应用[J]. 中国铁道科学,2019,40(3): 80-88. doi: 10.3969/j.issn.1001-4632.2019.03.12CHENG Di, HU Xiaoyi, LIU Fengshou, et al. Evaluation method and application of wheel-rail profile matching for high speed train based on improved analytic hierarchy process[J]. China Railway Science, 2019, 40(3): 80-88. doi: 10.3969/j.issn.1001-4632.2019.03.12 [17] WU L, LIU J Q, DHANASEKAR M, et al. Optimisation of railhead profiles for curved tracks using improved non-uniform rational B-splines and measured profiles[J]. Wear, 2019, 418/419: 123-132. doi: 10.1016/j.wear.2018.11.012 期刊类型引用(4)
1. 孟凡愚,沈龙江,邓小星,姚远. 考虑等效锥度离散度的JM_3车轮型面优化. 西南交通大学学报. 2025(02): 346-355 .  本站查看
本站查看2. 曾鲁庆,崔大宾,李立. 基于机电液耦合的钢轨打磨模型. 西南交通大学学报. 2025(02): 364-373 .  本站查看
本站查看3. 廖建文,香海杰. 轨道润滑的智能运维技术. 轨道交通装备与技术. 2023(02): 7-11 .  百度学术
百度学术4. 张斌,汪龙洋,杨宗超,孙奇,万文超,汤金伟. 小半径曲线段钢轨打磨方案比选及动力特性研究. 振动与冲击. 2023(24): 169-174+184 .  百度学术
百度学术其他类型引用(9)
-





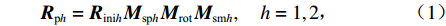
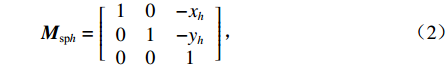
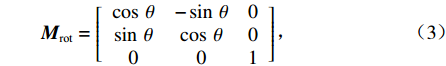
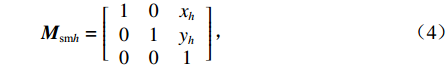


 下载:
下载:
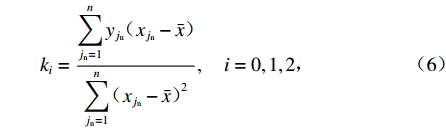
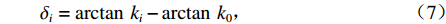
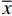






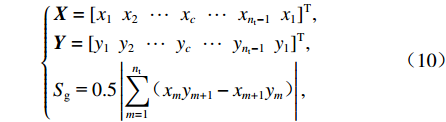

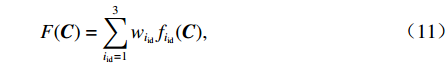
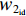
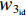
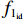
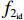
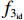

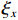

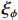



 下载:
下载:


 百度学术
百度学术