Analysis of Aggregate Interaction Based on Configuration Force Fracture Criterion
-
摘要:
为研究沥青混凝土内部裂纹初始构型(如裂纹初始偏转角,空间位置)的改变对裂纹扩展路径以及裂纹扩展方式的影响,以裂尖构型力为断裂准则,通过扩展有限元XFEM建立具有不同初始构型的裂纹模型,模拟裂纹经过单骨料和非对称双骨料的情况;从裂纹扩展路径和裂尖构型力变化等方面分析骨料干涉作用下裂纹初始构型对裂纹扩展的影响. 结果表明:1) 在单骨料干涉作用下,裂尖构型力随骨料与初始裂尖夹角的增大而逐渐增大,表明骨料对裂纹扩展的干涉作用逐渐减弱,当其超过60° 后,骨料对裂纹扩展的干涉作用可忽略不计;2) 在非对称双骨料干涉作用下,随着骨料圆心连线与
x 轴夹角的增大,双骨料对裂纹扩展干涉作用愈加明显,当其大于45° 时,裂尖构型力明显偏小,即骨料对裂纹扩展表现出“止裂”效果;3) 当裂纹初始偏转角发生改变时,单骨料与非对称双骨料对裂纹扩展的干涉作用具有相似性,其裂尖构型力随偏转角增大呈现先增加后减小的趋势;4) 当偏转角为45° 时,裂尖构型力偏大,意味着裂纹趋于非稳定状态,骨料对裂纹扩展的抑制效果较弱,致使骨料对沥青混合料抗裂性能的提高受到一定限制.Abstract:In order to study the influence of the initial crack configuration (such as the initial crack deflection angle and the space position) in asphalt concrete on the change of crack propagation path and growth mode, A crack model with different initial configurations was established by extended finite element XFEM with configuration force at crack tip as fracture criterion to simulate the crack propagation through single aggregate and asymmetric double aggregate. The influence of initial crack configuration on crack propagation was analyzed from the aspects of crack propagation path and crack tip’s configurational force. The results are summarized below: 1) Under single aggregate interference, the crack tip’s configurational force gradually increases with the increase of the angle (the angle between the line connecting the crack tip and the center of the aggregate and the
x -axis), indicating that the interference effect of the aggregate on the crack propagation gradually weakens. When the angle is over 60°, the interference effect of aggregate on crack propagation is negligible; 2) Under the interference of asymmetric double aggregate, as the angle (the angle between the line connecting the center of the aggregate and thex -axis) increases, the aggregate interference effect on crack propagation gradually enhances. When the angle is over 45°, the crack tip’s configurational force is significantly smaller, that is, the aggregate exhibits a “crack arresting” effect on the crack propagation; 3) When the initial crack deflection angle changes, the interference effect of single aggregate and asymmetric duel-aggregate on crack propagation is similar. The crack tip’s configuration force increases first and then decreases with the increase of deflection angle ; 4) When it is equal to 45°, the larger crack tip’s configurational force means that the crack tends to be in an unstable state, and the inhibitory effect of the aggregate on crack propagation is weak; hence, the improvement of the crack resistance of the asphalt concrete by the aggregate is limited. -
在细观尺度上,沥青混凝土内部通常存在着空隙、裂纹等缺陷. 在荷载作用下,沥青混凝土内部裂纹缺陷不断扩展形成宏观裂缝,最终导致沥青混凝土宏观力学特性的丧失. 国内外学者针对沥青混凝土裂纹扩展问题,开展了理论、数值以及试验相关研究:Atkinson等[1-3]采用包含夹杂的裂纹尖端应力强度应力因子的数值计算方法,研究分析了宏观裂纹和微观裂纹缺陷的相互作用机制,并在此基础上提出了裂纹表面相互干涉的有限元数值计算方法;Mishuris等[4]采用数值模拟的方法,研究分析了裂纹和多缺陷介质的相互作用机制;毛成等[5]采用数值计算与室内试验结合的方法,从宏观角度研究分析了多相夹杂体内部宏观偏直裂纹的扩展规律;黄晓明等[6]则基于裂纹局部衰坏区模型,提出了沥青混凝土内部裂纹稳定扩展阶段开裂速率的力学模型. 然而,从沥青混凝土细观结构上来看,其内部骨料(硬夹杂)会使裂尖域内的应力场发生变化,从而导致裂尖构型力、裂纹扩展路径以及裂纹扩展方式等具有明显差异. 郭荣鑫[7]基于Eshelby等效夹杂理论研究发现,空隙和骨料对裂纹扩展的干涉机制存在明显的不同. 束一秀等[8]采用XFEM方法,研究分析了裂纹扩展过程中,硬夹杂对裂纹扩展路径以及裂尖应力强度因子的影响.
尽管传统的断裂力学已经广泛应用于裂纹与夹杂的干涉问题研究中,但是由于断裂现象涉及外部载荷、裂纹构型等各方面因素,断裂准则仍存在一定局限性:能量释放率G、J 积分、裂纹尖端应力强度因子K和张开位移CTOD仅可以用来预测裂纹的孕育或起裂,而无法预测裂纹的扩展方向;最大切向应力MTS和应变能密度因子S 虽然能够有效预测裂纹起裂方向,但切向应力的计算必须沿着距裂纹尖端的临界距离
rc 进行,rc 的大小会干扰裂纹扩展的预测结果,而S则通过求解一类分支裂纹的边值问题得到复合裂纹能量释放率的真值,且分支裂纹能量释放率必须趋于0.近年来研究发现, 当材料中缺陷(夹杂、空穴、位错、裂纹、局部塑性变形区等) 的构型(尺寸、形状和位置) 改变时,会引起材料自由能的变化,驱使材料构型变化的力称为构型力,而与裂纹扩展相关的驱动力可用裂尖构型力表示,并提出了最大等效构型应力作为断裂准则预测复合型裂纹扩展[9]. 因此,基于材料空间上发展起来的材料构型力学(MCM) 为解决复合型裂纹扩展等复杂缺陷问题提供了新的思路[10]. 国内外学者将构型力概念与扩展有限元方法结合,分别以脆性材料[11]、黏弹性材料[12]、超弹性材料[13]为研究对象,通过模拟裂纹扩展问题,研究分析了裂纹扩展过程中的能量耗散与裂尖构型力之间的关系. 同时,国内学者以裂尖构型力的角度,阐述了空隙对裂纹扩展的干涉作用机理[14-15].
综上,相关研究多集中采用传统断裂力学准则研究空隙对裂纹扩展的干涉作用,但由于空隙和骨料对裂纹扩展的干涉机制存在明显不同,因此,骨料对裂纹扩展的干涉作用仍不可忽略. 本文基于构型力断裂准则,通过扩展有限元方法(XFEM)建立−20 ℃条件下裂纹扩展模型,从裂纹扩展路径,裂尖构型力等角度分析不同裂纹初始构型条件下,骨料对裂纹扩展的干涉作用.
1. 复合型裂纹扩展模型
1.1 基于材料构型力学的断裂准则
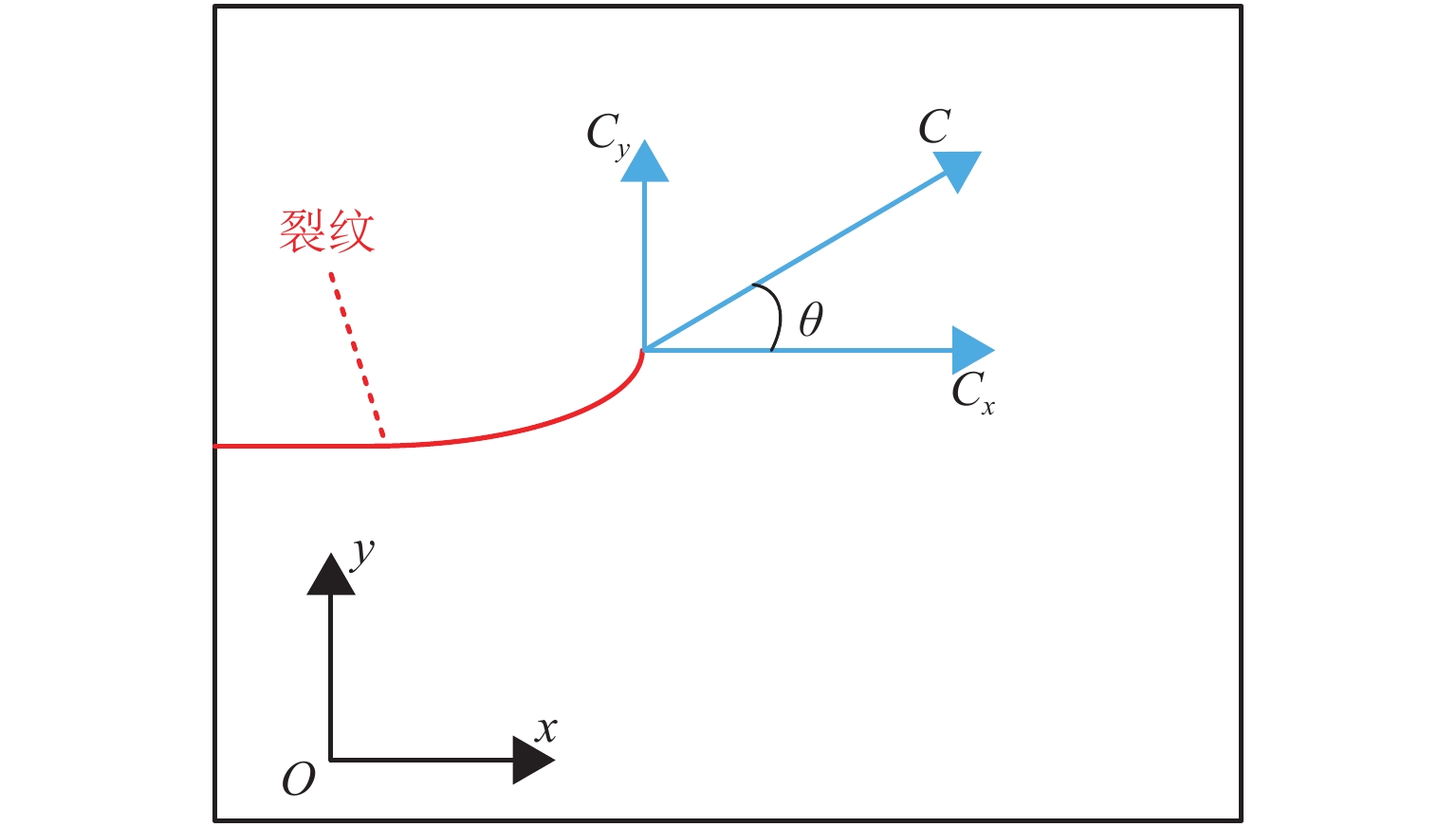
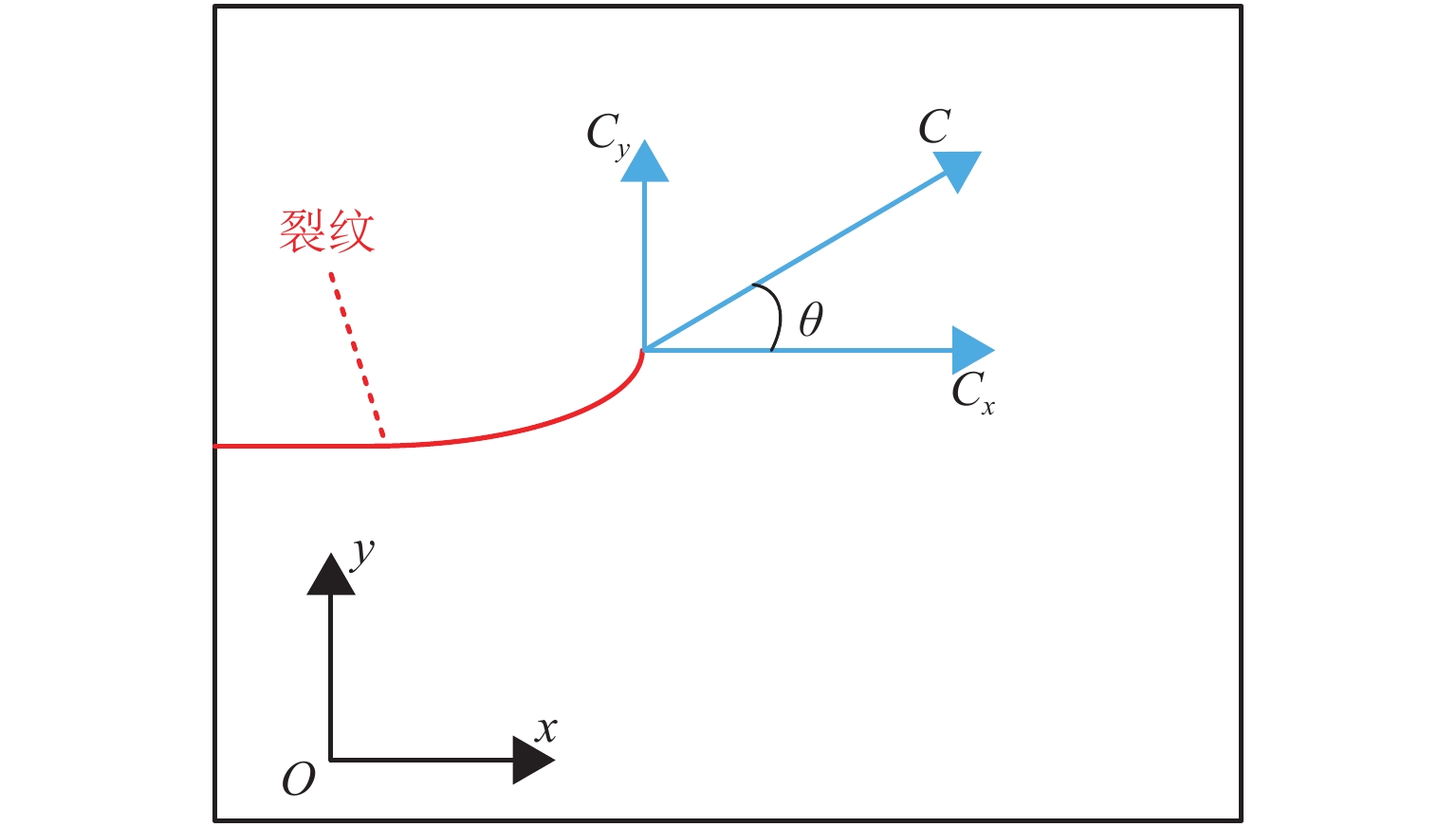
基于构型力学理论建立相应的断裂准则不需要定义裂尖的断裂进行区临界距离,即裂尖的塑性损伤区,因而可以较为准确地预测裂纹起裂;同时能够描述材料中任意裂纹的起裂和扩展方向,其断裂准则如图1所示,图中:C为构型力,与材料属性及应力强度因子相关;Cx、Cy分别为材料构型力在x轴和y轴方向的分量,可由应变能密度W对x和y的显式求导得到;θ为裂纹断裂角.
1) 当裂纹尖端的扩展驱动力大于材料的断裂韧性,裂纹发生扩展,如式(1).
C=√C2x+C2y⩾Cth, (1) 式中: Cth为材料的临界构型力断裂韧性,为材料常数,与裂纹构型和荷载无关,可通过数字散斑试验获得[16].
{Cx=−(∂W∂x)expl,Cy=−(∂W∂y)expl. (2) θ=arctanCyCx. (3) 结合式(2)、(3),可通过数值计算裂纹扩展时裂纹尖构型力的变化. 当C > Cth时,裂纹发生扩展,并根据式(3)计算θ,用于建立扩展后的几何模型,并以扩展后的裂纹尖端作为新的裂纹尖端.
在数值模拟过程中,为了加快运算的速度,一方面采用Advancing front方法对裂纹可能会通过的区域采用局部加密的自由网格进行处理,并仅对裂纹尖端附近的网格进行重新划分,而由于远离裂尖的区域对计算结果影响不大,则采用固定网格划分;另一方面,为避免裂尖域内应力场出现奇异性现象,将沥青混合料内部骨料颗粒视为圆形颗粒.
1.2 模型构建
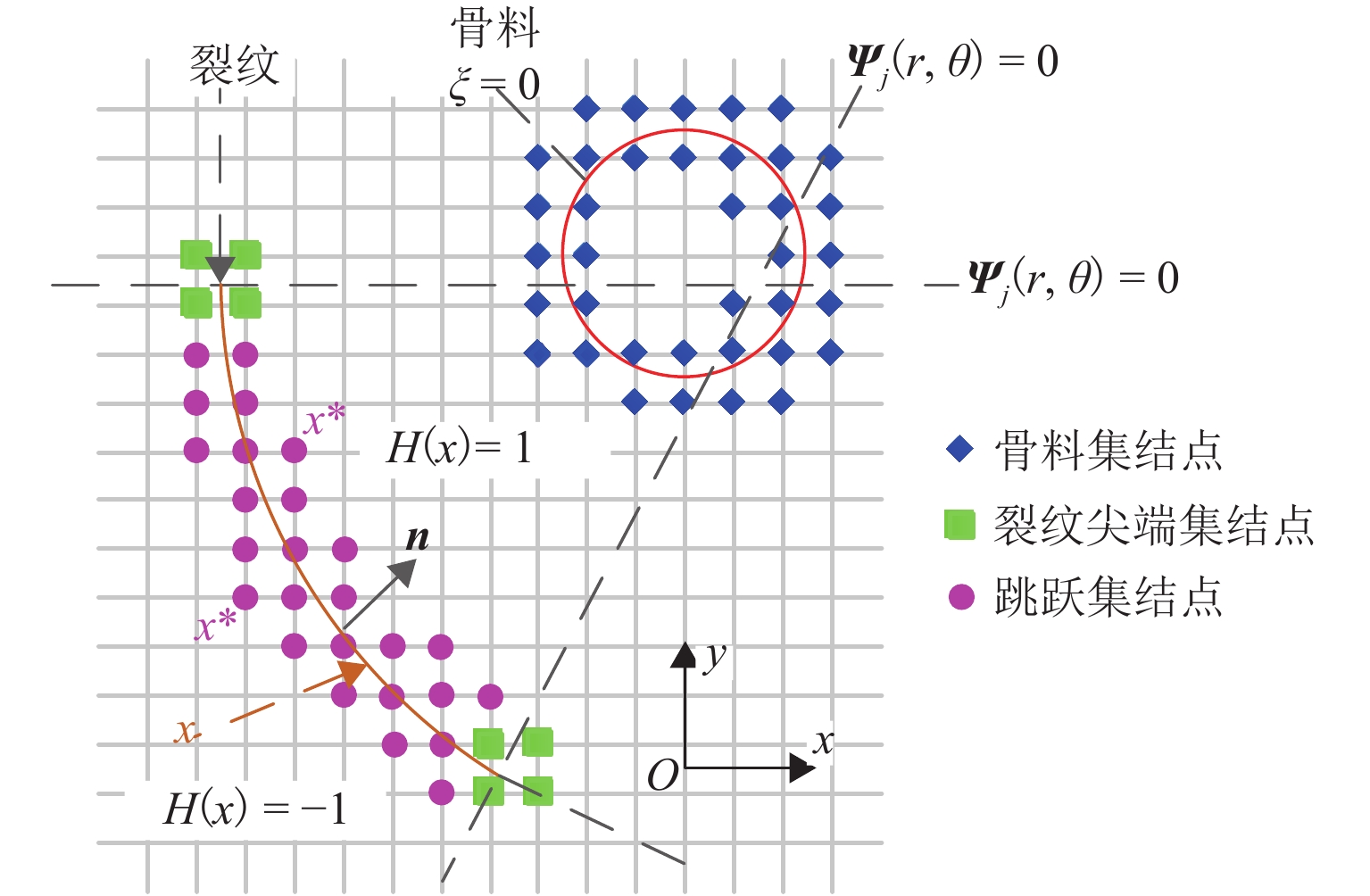
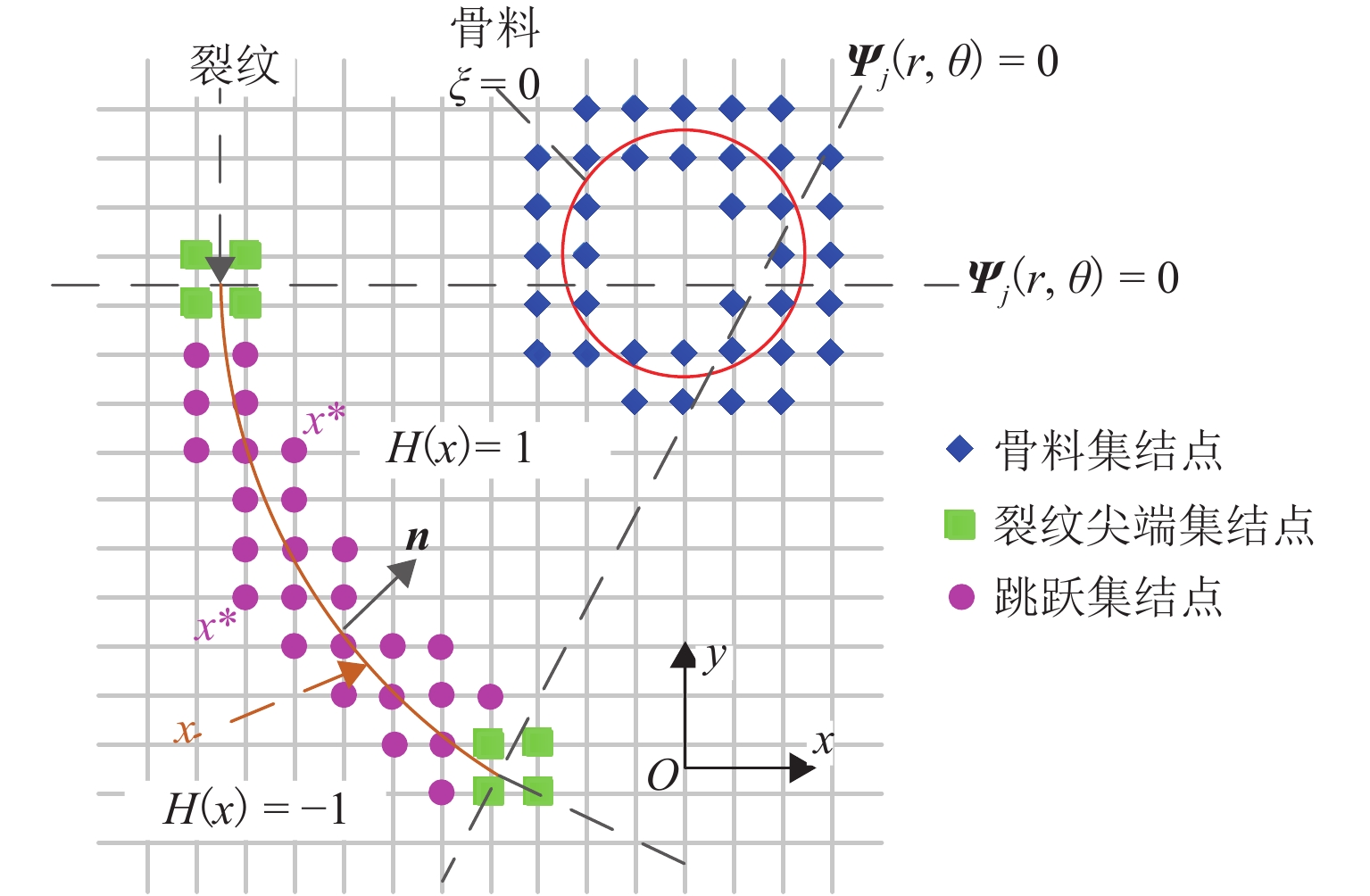
对于沥青混合料细观结构而言,其内部夹杂体包括空隙和粗骨料. 对于包含裂纹和夹杂体的区域,如图2所示,图2中:x为裂纹面上的样本点;x*为裂纹穿过的单元的节点;n为单位外法线向量;H(x)为跳跃函数,表征裂纹面处位移跳跃性;ξ为符号距离函数;Ψj(r,θ)为渐进函数,表征裂纹尖端附近应力奇异性. 其单元内任意点位移u可以用节点位移表示[17],如式(4).
\begin{split} & \boldsymbol u = \sum\limits_i {{N_i}} (x){{\boldsymbol{u}}_i} + \sum\limits_i {{N_i}} (x)\phi {{\boldsymbol{a}}_i} + \sum\limits_i {{N_i}} (x)H(x){{\boldsymbol{b}}_i} + \\ &\quad \sum\limits_i \left({{N_i}} (x)\sum\limits_j {{{\boldsymbol{\varPsi}} _j}} (r,\theta ){{\boldsymbol{c}}_{ji}}\right) , \\[-13pt] \end{split} (4) 式中:ui为单元内任意一点的位移向量;Ni(x)为形状函数; ϕ为扩展函数,表征夹杂位置和几何特征; ai、bi、cji分别为各自节点所对应的节点位移.
由于裂纹属于非闭合曲线,可用H(x)和Ψj(r,θ)分别表征裂纹的位置以及几何特征[18],如式(5)、(6).
H(x)=\left\{\begin{array}{l} 1\text{,}\quad (x-x^*){\boldsymbol{n}}\geqslant 0,\\ -1\text{,}\quad\; 其他,\end{array}\right. (5) \begin{split} & {{\boldsymbol{\varPsi}} _j}(r,\theta ) = \left( \sqrt r \cos \;\frac{\theta }{2},\sqrt r \sin \;\frac{\theta }{2},\sqrt r \cos\; \frac{\theta }{2}\sin\; \theta, \right.\\ &\left.\quad \sqrt r \cos\; \frac{\theta }{2}\sin \;\theta \right). \end{split} (6) 同时, ϕ可表述为[18]
\phi = \sum\limits_i {{N_i}} (x)\left| {{\xi _{}}} \right| - \left| {\sum\limits_i {{N_i}} (x)\xi } \right| . (7) 由于沥青混合料内部骨料的边界为封闭曲线,因此,仅需符号距离函数ξ来描述其位置和几何特征. Eshelby等效夹杂理论[19]中,通常将夹杂体视为椭圆形夹杂,即ξ如式(8),当ξ=0时,即可得到骨料的轮廓.
\xi = {\left( {\frac{{{x_1}}}{a}} \right)^2} + {\left( {\frac{{{y_1}}}{b}} \right)^2} - 1 , (8) 式中:(x1,y1)分别为骨料边界坐标;a、b为常数,当a=b时,骨料轮廓线为圆形,如图2所示.
综上,借助扩展有限法(XFEM)建立沥青混合料细观结构模型,其细观非连续性可由与额外自由度相关联的扩展函数确定;同时,由于裂纹体完全独立于网格,因此,在裂纹扩展过程中不需要进行网格的重新划分.
1.3 材料参数设置
基于室内低温试验研究成果[20],本次数值计算以空隙率为2%的AC-13级配沥青混凝土为算例,级配如表1所示.
表 1 AC-13沥青混凝土级配Table 1. Gradation of AC-13 Asphalt Concrete筛孔尺寸/mm 16.000 13.200 9.500 4.750 2.360 1.180 0.600 0.300 0.150 0.075 通过率/% 100.0 97.5 84.0 62.5 42.5 32.0 24.0 15.5 11.0 6.0 根据以往研究成果[21],在−20 ℃下,AC-13级配沥青混凝土相关材料参数如表2所示.
表 2 −20 ℃下AC-13级配沥青混凝土材料参数Table 2. Parameters of AC-13 asphalt concrete at −20 ℃材料相类 计算参数 数值 骨料 弹性模量 E/GPa 55.5 抗拉强度 σ/MPa 27.60 沥青混合料 抗拉强度 σ/MPa 3.55 断裂能/(J·m−2) 275 本例计算中,着重研究裂纹初始构型改变时,骨料对裂纹扩展的干涉作用,因此,可不考虑裂纹初始构型对失效临界荷载的影响,即本例计算不涉及失效临界荷载的判定. 由表2可知,沥青混合料抗拉强度σ=3.55 MPa,故当外界载荷(4 MPa)≥3.55 MPa时,沥青混合料发生破坏,即内部裂纹发生扩展,意味着其内部裂纹扩展始终满足C≥Cth的裂纹起裂条件. 基于此,本例计算确定施加载荷为4 MPa.
2. 裂纹与骨料的相对位置
本例以2.36 mm粒径的粗骨料为算例,以骨料为中心,通过扩展有限元建立沥青混凝土局部RVE细观模型,研究分析裂纹与骨料的相对位置发生改变时,骨料对裂纹扩展干涉作用的变化.
2.1 单骨料干涉作用
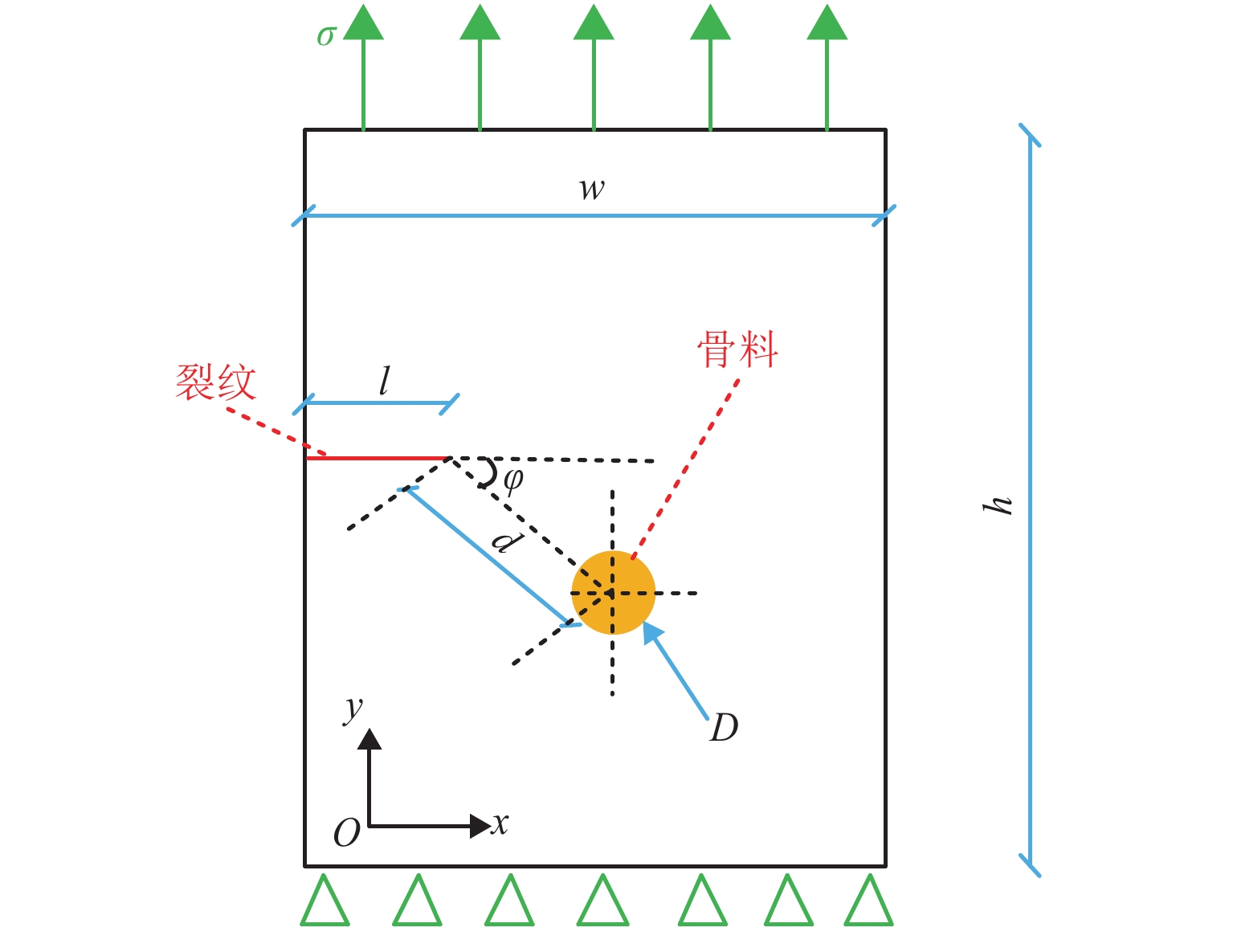
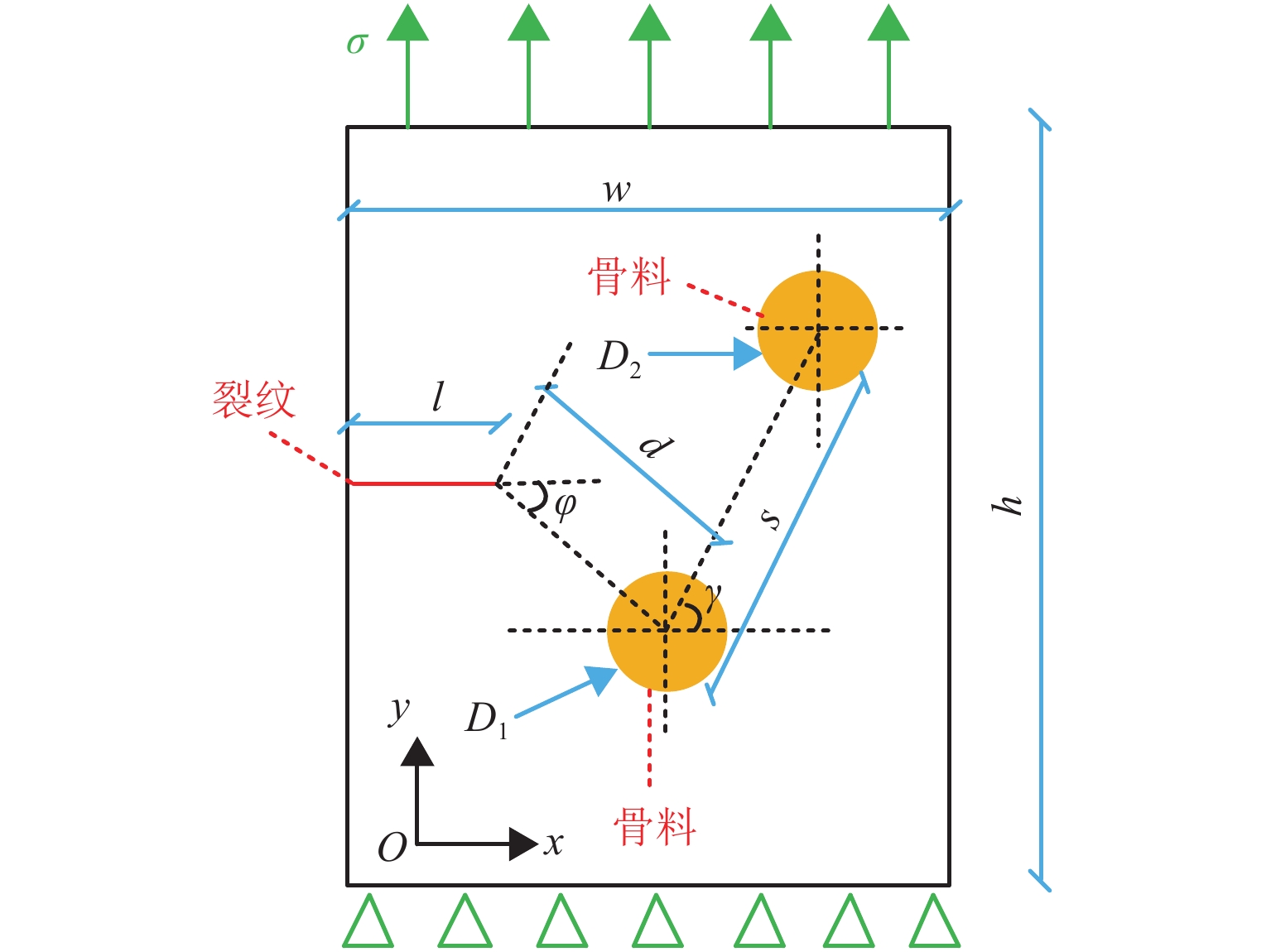
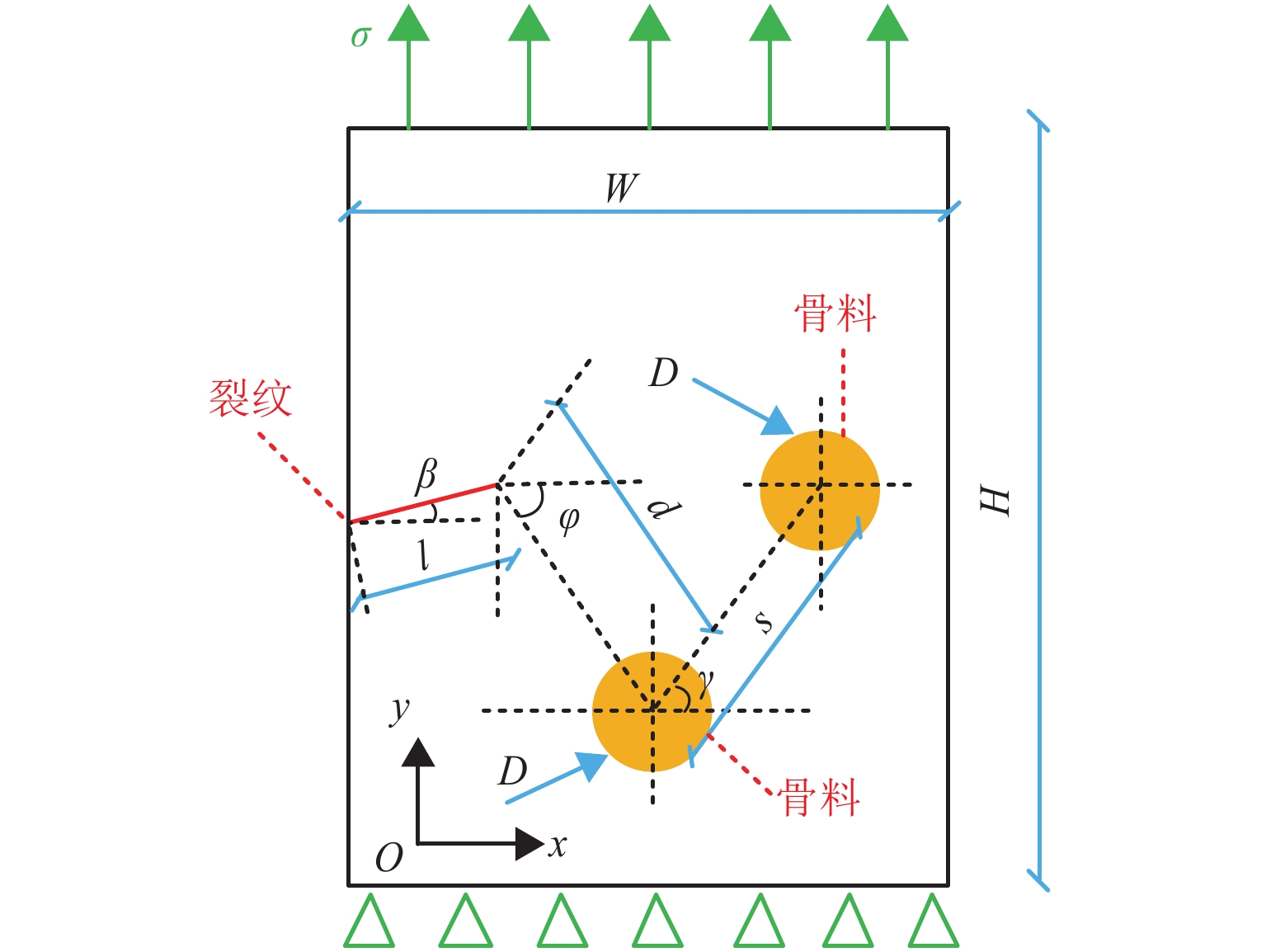
本节建立的扩展有限元模型中,裂纹与就近的骨料相对位置可以通过裂尖与骨料圆心连线与x轴夹角φ和圆形距裂尖的距离d表示,模型如图3,图中参数设置如表3所示.
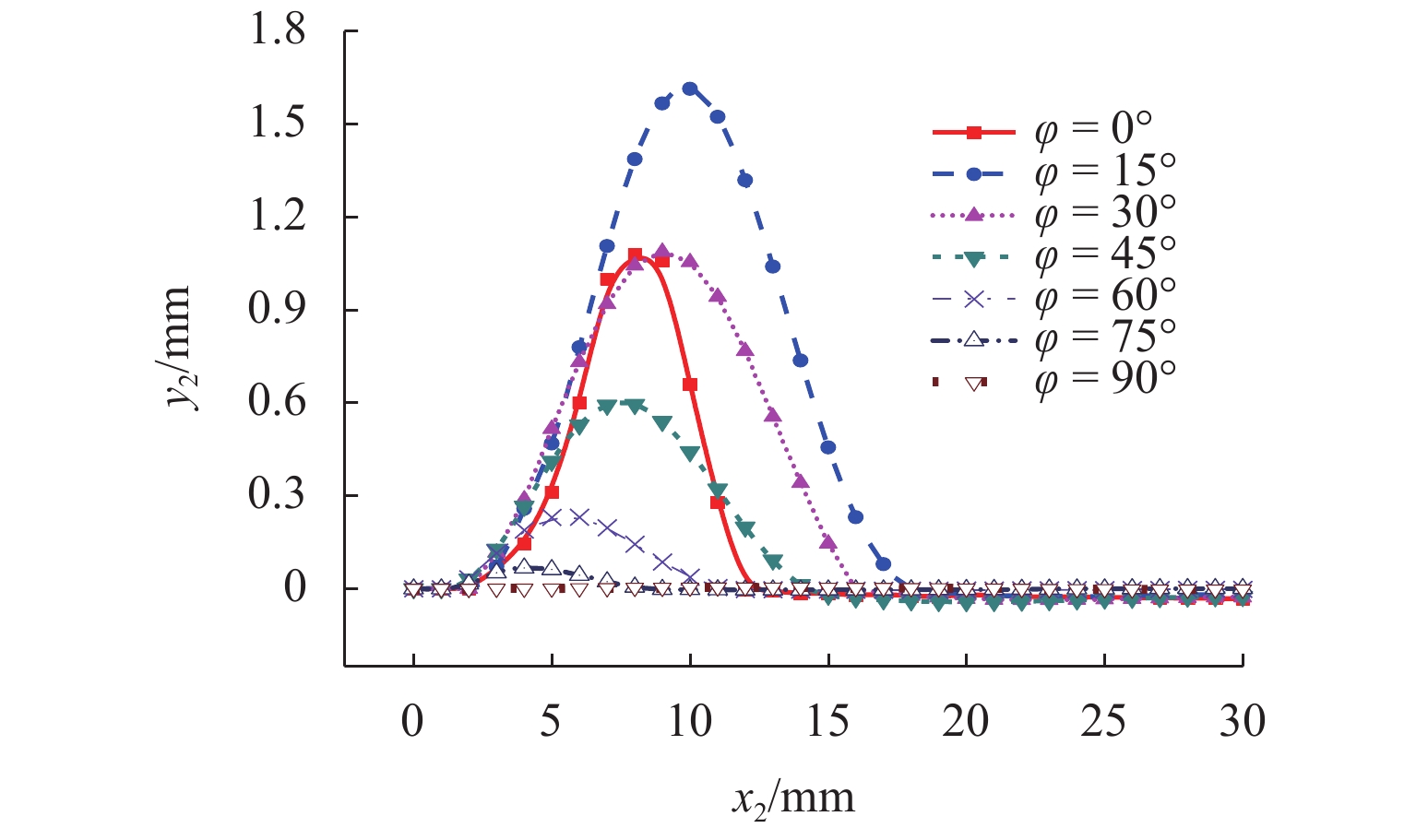
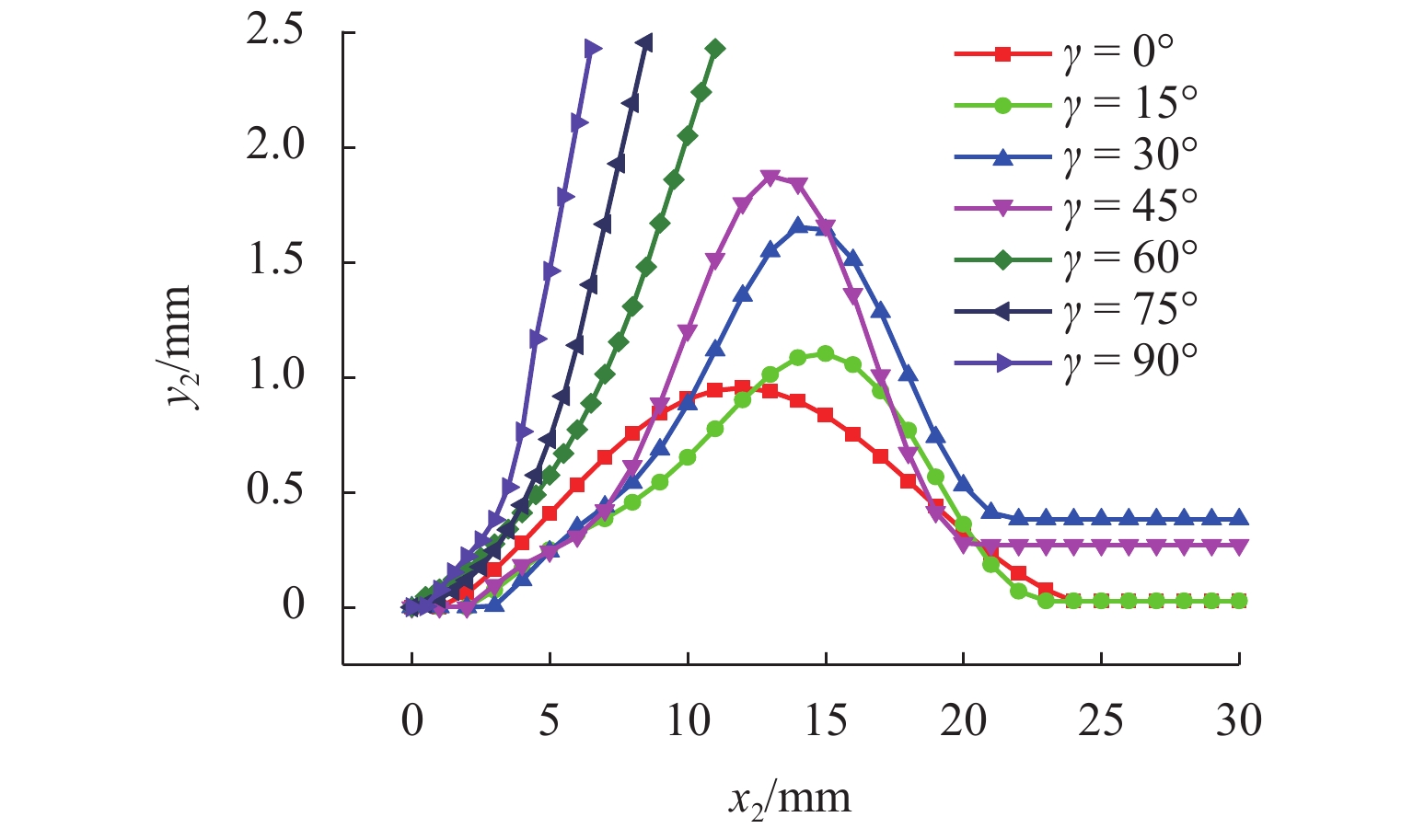
表 3 扩展有限元模型设置参数Table 3. Parameters of extended finite element model材料类型 参数类型 数值 沥青混合料 宽度 W/mm 30 长度 H/mm 40 裂纹 裂纹长度 l/mm 2 粗骨料 粒径 D/mm 2.36 d/mm 8 夹角\varphi /(°) 0、15、30、45、60、75、90 由数值计算结果,以初始裂尖坐标为坐标原点,可以提取裂纹扩展过程中裂尖坐标(x2, y2)变化曲线,即裂纹扩展路径,如图4所示.
通过图4可以得出:当骨料与初始裂尖的夹角φ < 45° 时,裂纹扩展受到骨料的强烈干涉作用;而当骨料与初始裂尖的夹角φ超过45° 时,骨料对裂纹扩展的影响非常小,呈现出近似Ⅰ型裂纹的趋势进行扩展;同时,裂纹在绕过骨料中心之后,骨料对裂纹扩展的干涉作用减弱,在纯拉伸状态下,以纯Ⅰ型裂纹形式发生扩展.
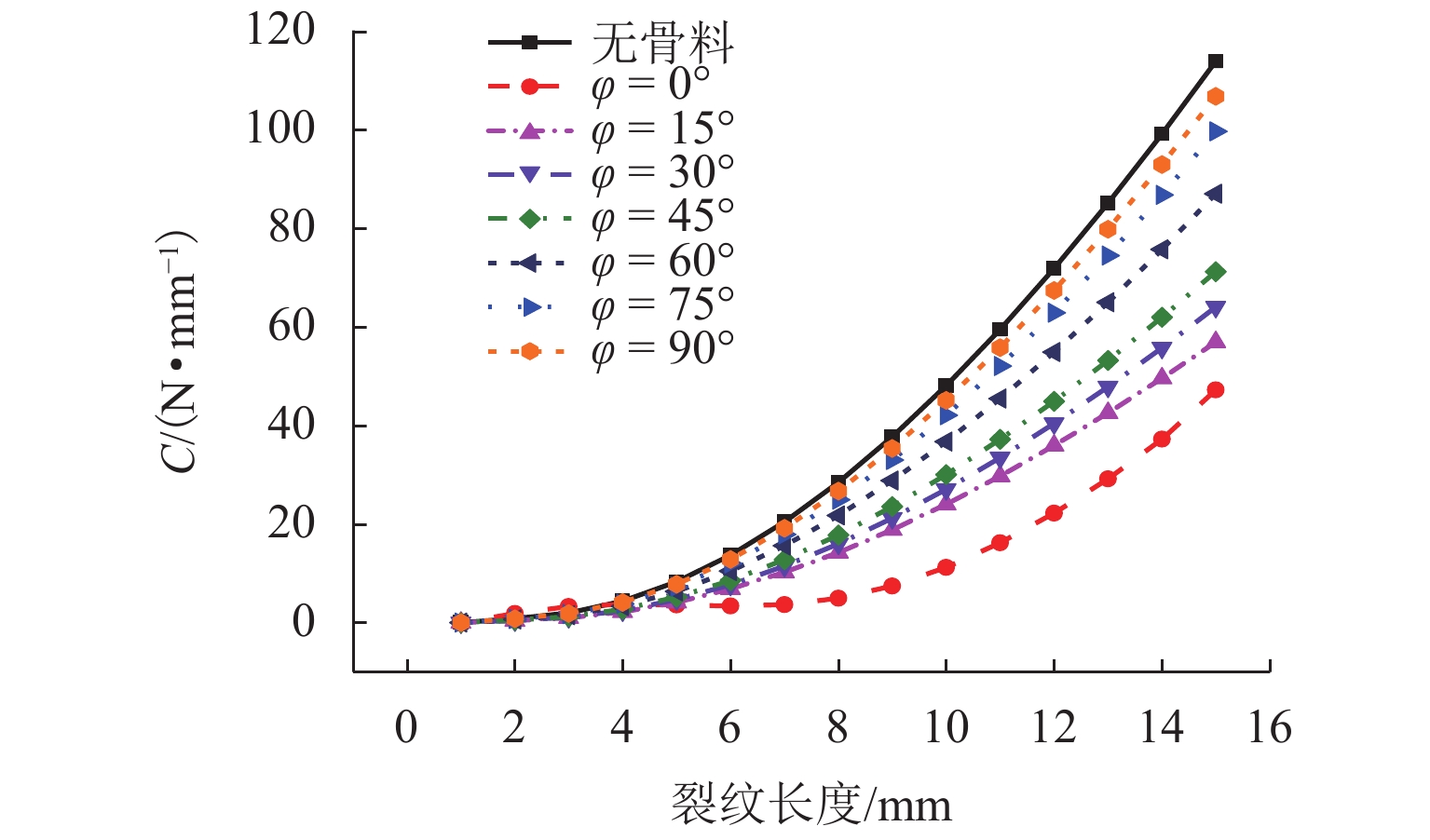
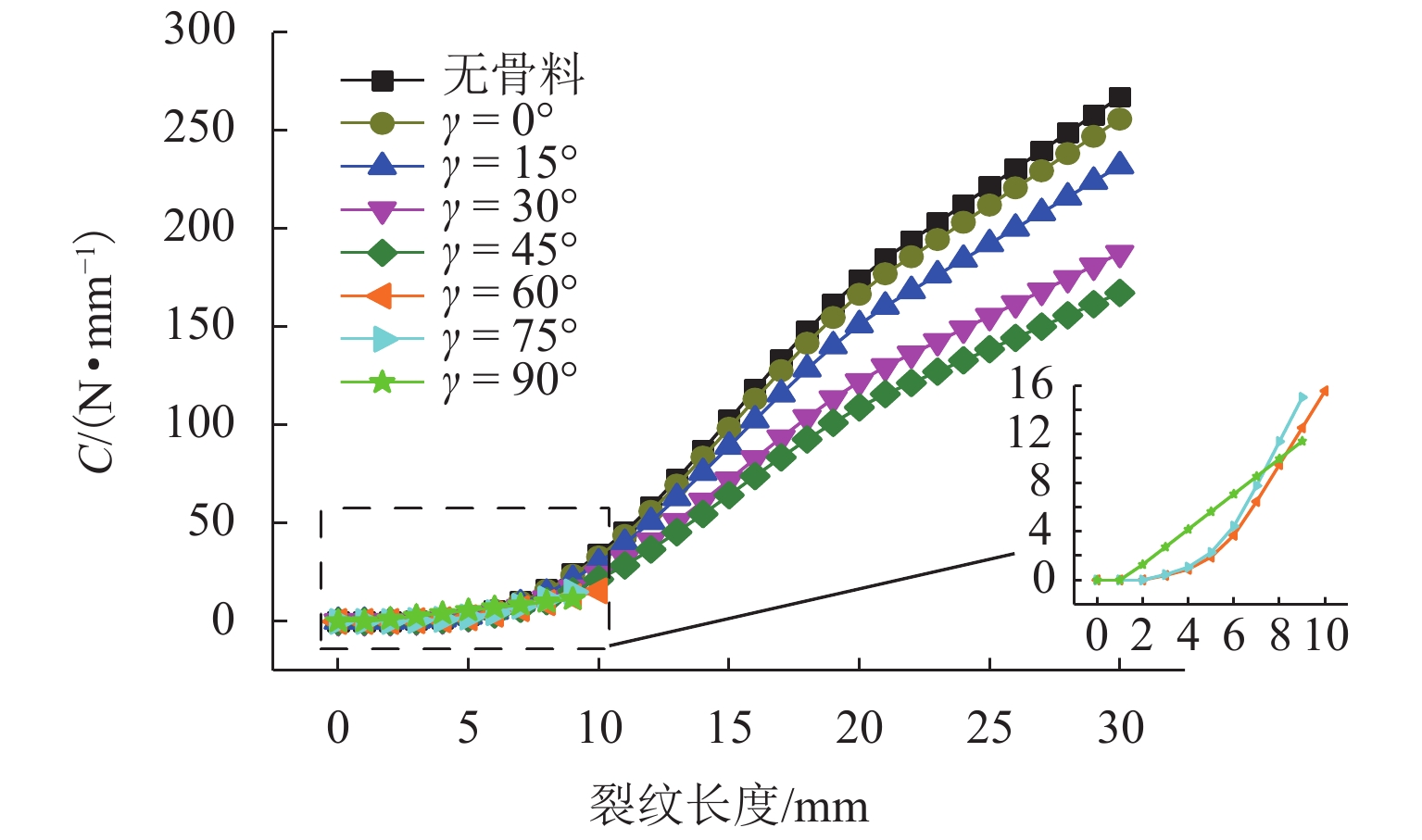
在扩展有限元计算中,裂尖只能停留在单元边界上,则可认为裂纹在单元内为一直线,因此,依据裂纹面场变量(PHILSM)计算结果,通过各个单元内部累积求和的方法获得整条裂纹长度. 基于此,仅提取骨料干涉作用下裂尖构型力随裂纹长度的变化. 为方便对比,对不含骨料的沥青砂浆在相同的边界条件下的裂尖构型力进行计算,裂尖构型力随裂纹长度的变化趋势如图5所示.
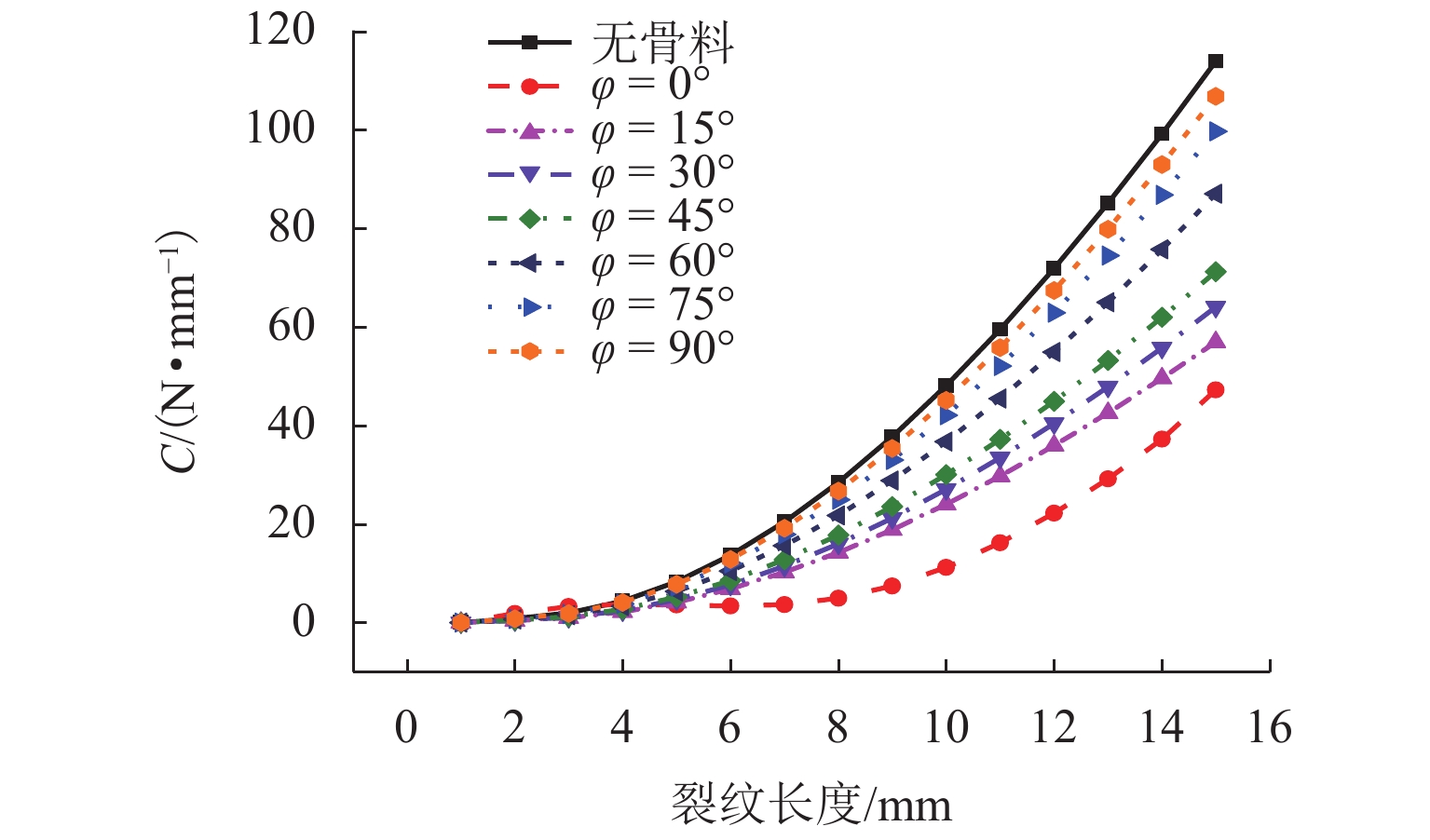
通过图5分析可知:当φ=0° 时,骨料能够明显抑制裂尖构型力的增加,直至裂纹与骨料发生聚合;裂尖构型力随着夹角φ的增大而增大,骨料对裂纹扩展的抑制作用逐渐减小;当φ≤45° 时,裂尖构型力一开始增加缓慢,之后又迅速增大,骨料对裂纹扩展具有强烈的干涉作用;当φ≥60° 时,裂纹从起裂就受到骨料的干涉作用,然而裂纹扩展时裂尖构型力与无骨料干涉状态下裂尖构型力相差不大,裂纹在起裂之后仅受到轻微的抑制作用,即当夹角φ≥60° 时,骨料对裂纹扩展的干涉作用可以忽略不计.
2.2 非对称双骨料干涉作用
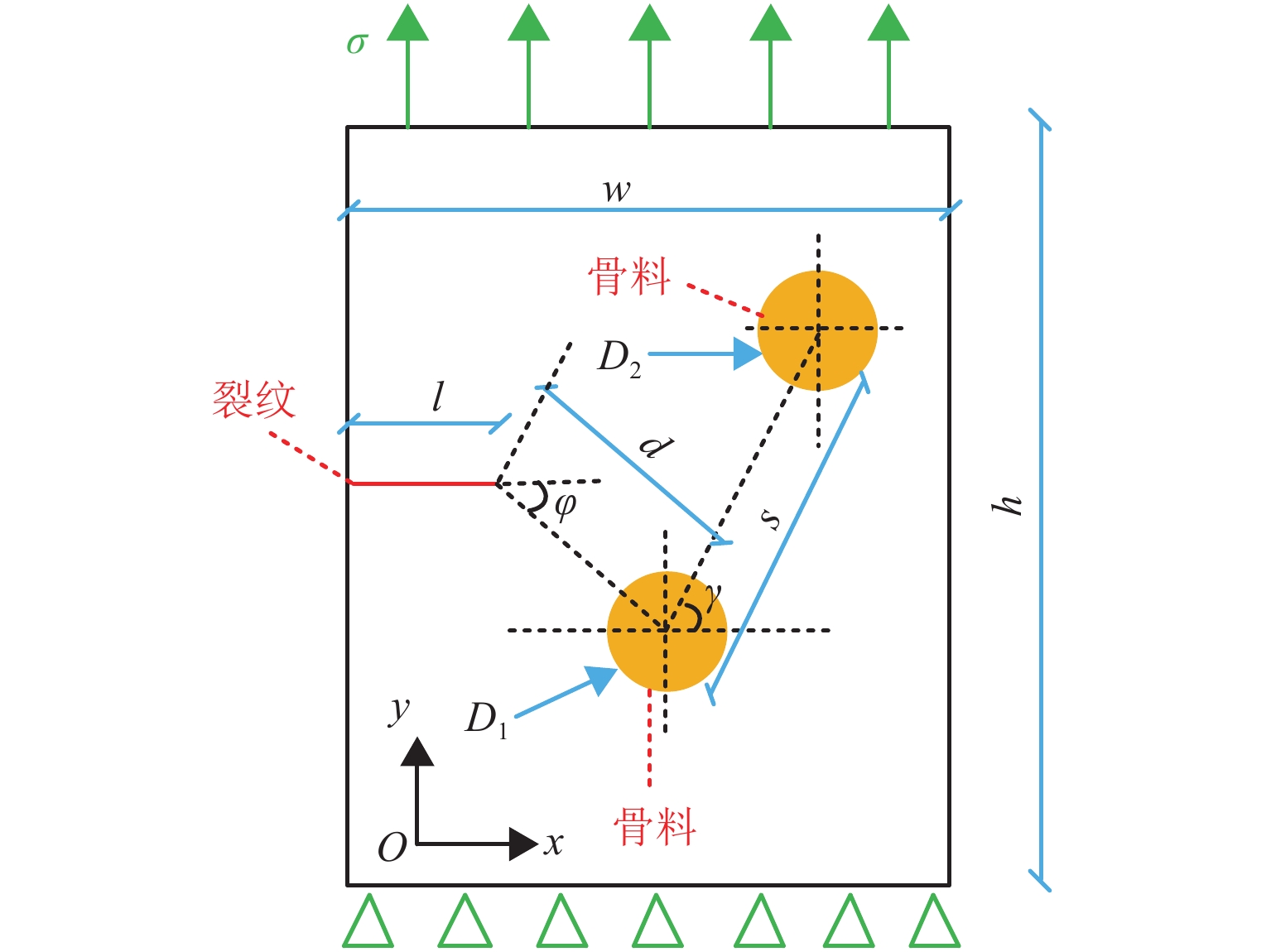
非对称双骨料扩展有限元模型尺寸与前节保持基本一致,模型中骨料间的相对位置以骨料圆心连线与水平轴的夹角γ以及圆心距s表示. 本节着重研究双骨料相对位置发生改变时,双骨料对裂纹扩展的干涉作用,本次以粒径为2.36 mm骨料为计算算例,第一个骨料与初始裂尖的夹角φ=45°,d=8 mm,双骨料圆心距s=8 mm,γ=0°,15°,30°,45°,60°,75°,90°,如图6所示,D1、D2分别为两个骨料的粒径.
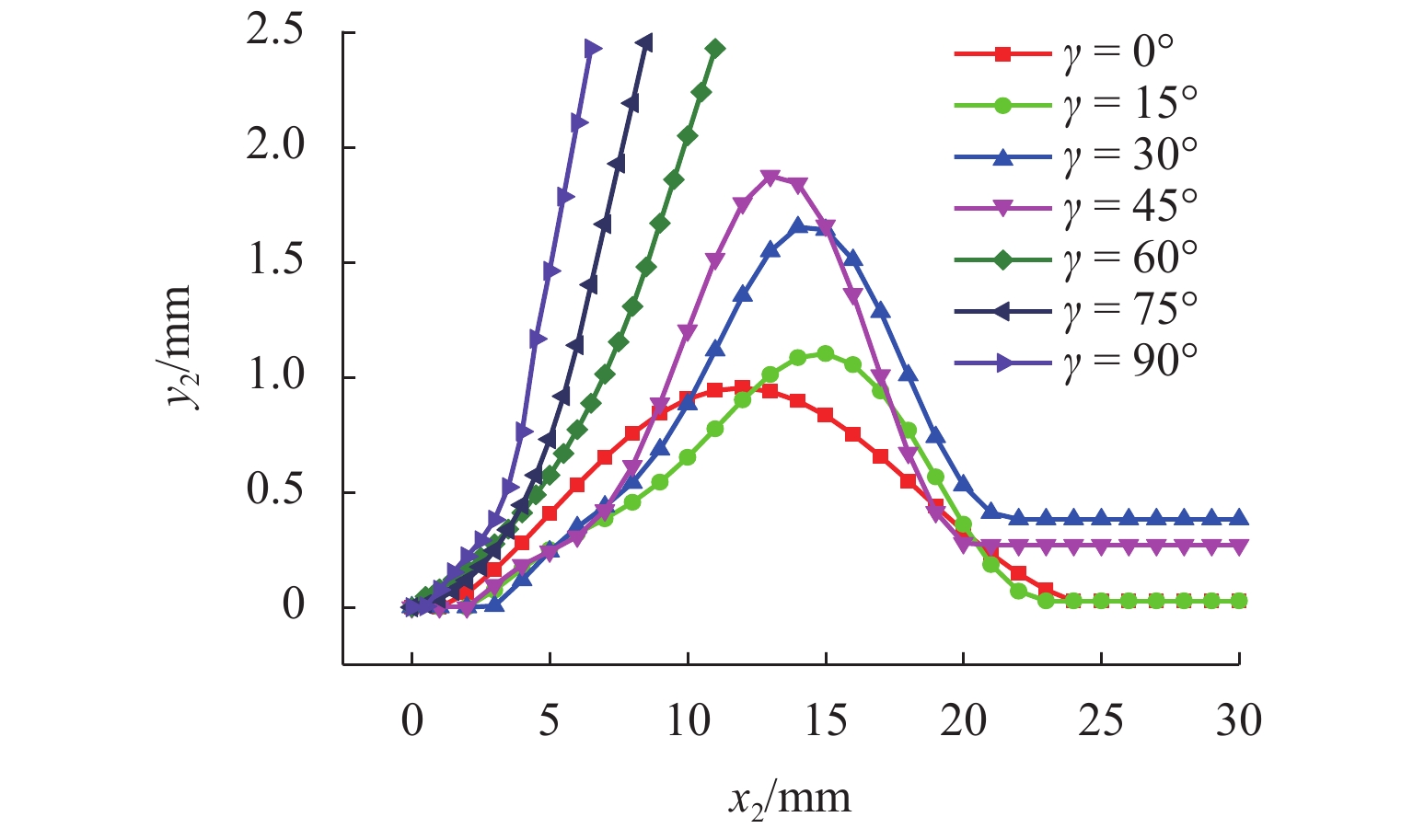
非对称双骨料干涉作用下,裂纹扩展过程如图7所示. 由图7可知:在纯拉伸荷载下,当γ≤45° 时,裂纹受到骨料的干涉作用而选择远离骨料的方向偏移,且随着
\gamma 的增大,双骨料对裂纹扩展干涉作用愈加明显,但是,当裂纹绕过第二个骨料中心后,骨料对裂纹的干涉作用减弱,裂纹以Ⅰ型裂纹形式继续扩展;当\gamma >45° 时,裂纹扩展受第一个骨料的干涉作用而发生偏离,直至与第二个骨料发生聚合,裂纹停止扩展.为方便对比,对不含骨料的沥青砂浆在相同的边界条件下的裂尖构型力进行计算,裂尖构型力随裂纹长度的变化趋势如图8所示.
由图8可知:裂尖构型力随着
\gamma 的增大而明显减小,说明骨料对裂纹扩展的抑制效果逐渐增强. 当骨料与初始裂尖的夹角\gamma = 0° 时,裂纹虽然受到骨料的干涉作用,但裂尖构型力与无骨料干涉状态下裂尖构型力相差不大,说明裂纹扩展仅受到双骨料的轻微干涉作用,即此时骨料对裂纹扩展的干涉可忽略不计.结合图7、8可知:当
\gamma >45° 时,由于裂纹扩展从起裂开始便受到第一个骨料的干涉作用而发生偏离,相对于第二个骨料而言,裂纹具有一定的初始偏转角β,在纯拉伸荷载作用下,裂纹与第二个骨料发生聚合,从而使第二个骨料对裂纹扩展表现出“止裂”效果,造成裂尖构型力大幅下降,说明当双骨料对裂纹扩展的干涉作用表现出“止裂”时,能够有效抑制裂尖构型力的增长,使裂纹趋于稳定状态,不易发生扩展,从而使得沥青混合料获得更好的抗裂性能;同时说明裂纹初始偏转角β在一定程度上影响着骨料对裂纹扩展的抑制效果.3. 裂纹初始偏转角β
3.1 单骨料干涉作用
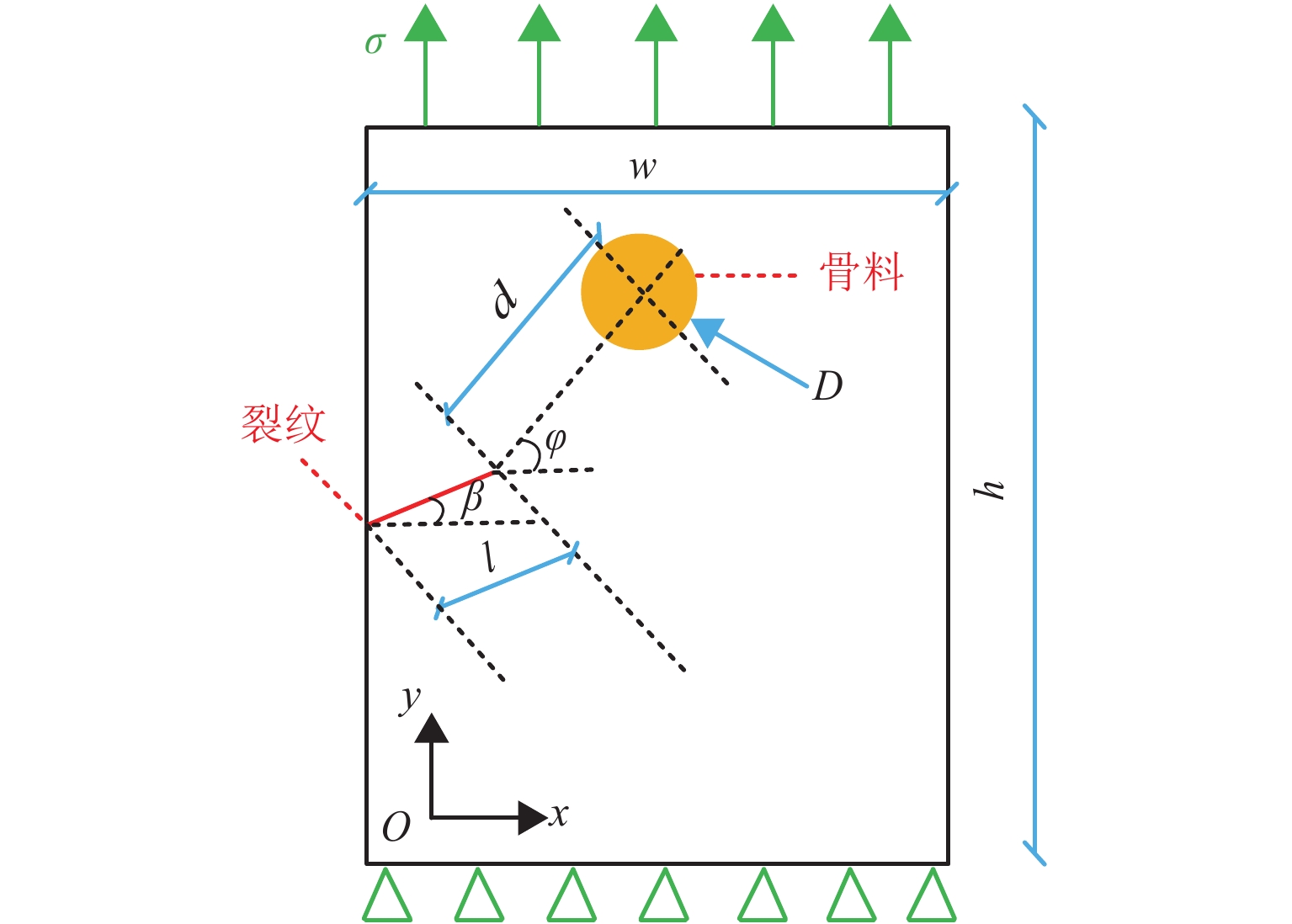
本节主要通过改变裂纹初始偏转角β研究分析骨料对裂纹扩展的干涉作用. 由前文可知,当γ>45° 时,骨料对裂纹扩展表现出“止裂”效果. 因此,本节着重于当裂纹初始偏转角发生改变时,骨料对裂纹“止裂”作用的变化. 其模型尺寸与边界条件及加载条件与前一节保持基本一致,如图9所示. 本次计算仍以2.36 mm骨料为计算算例,φ=60°,d=8 mm,β=0°,15°,30°,45°,60°,75°.
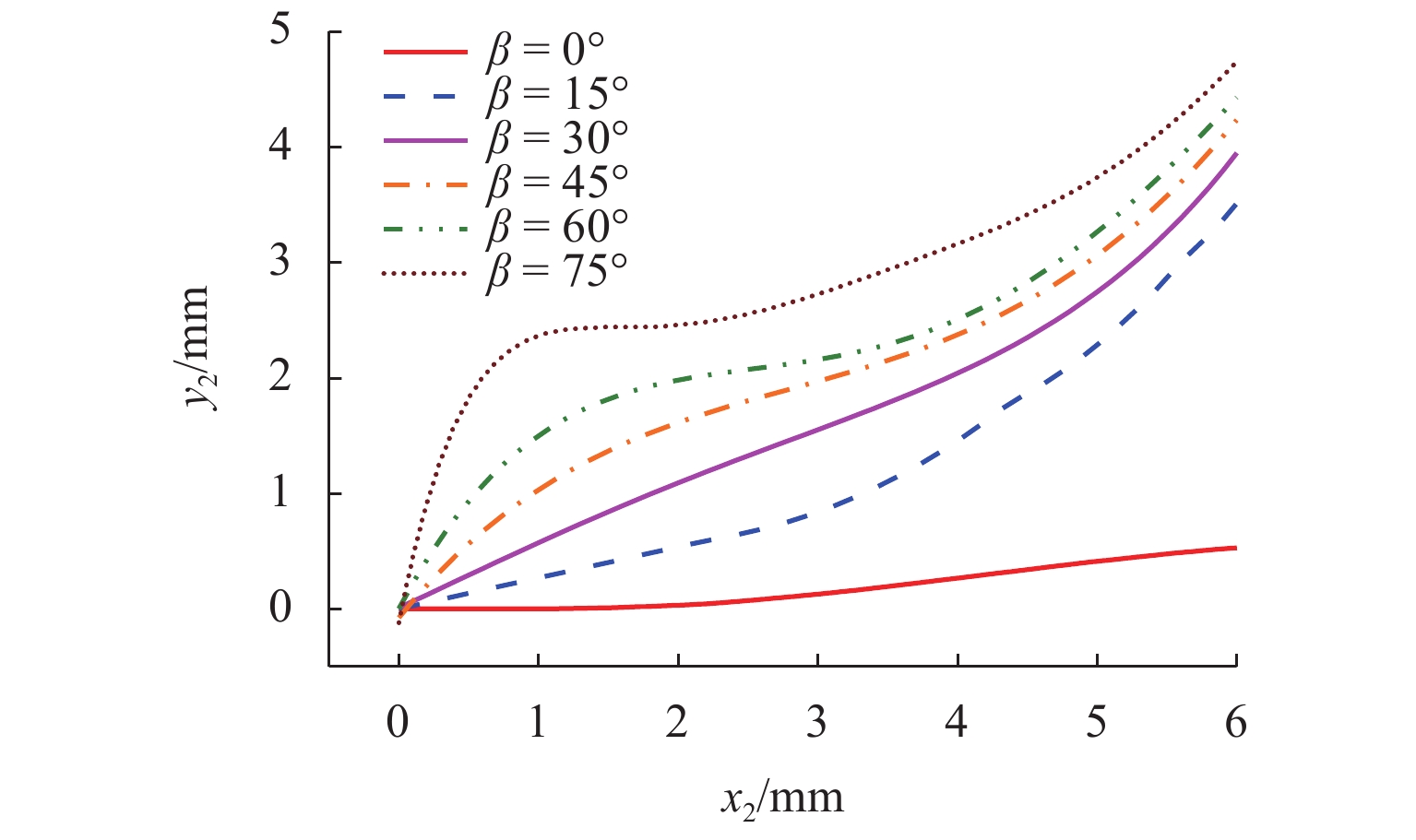
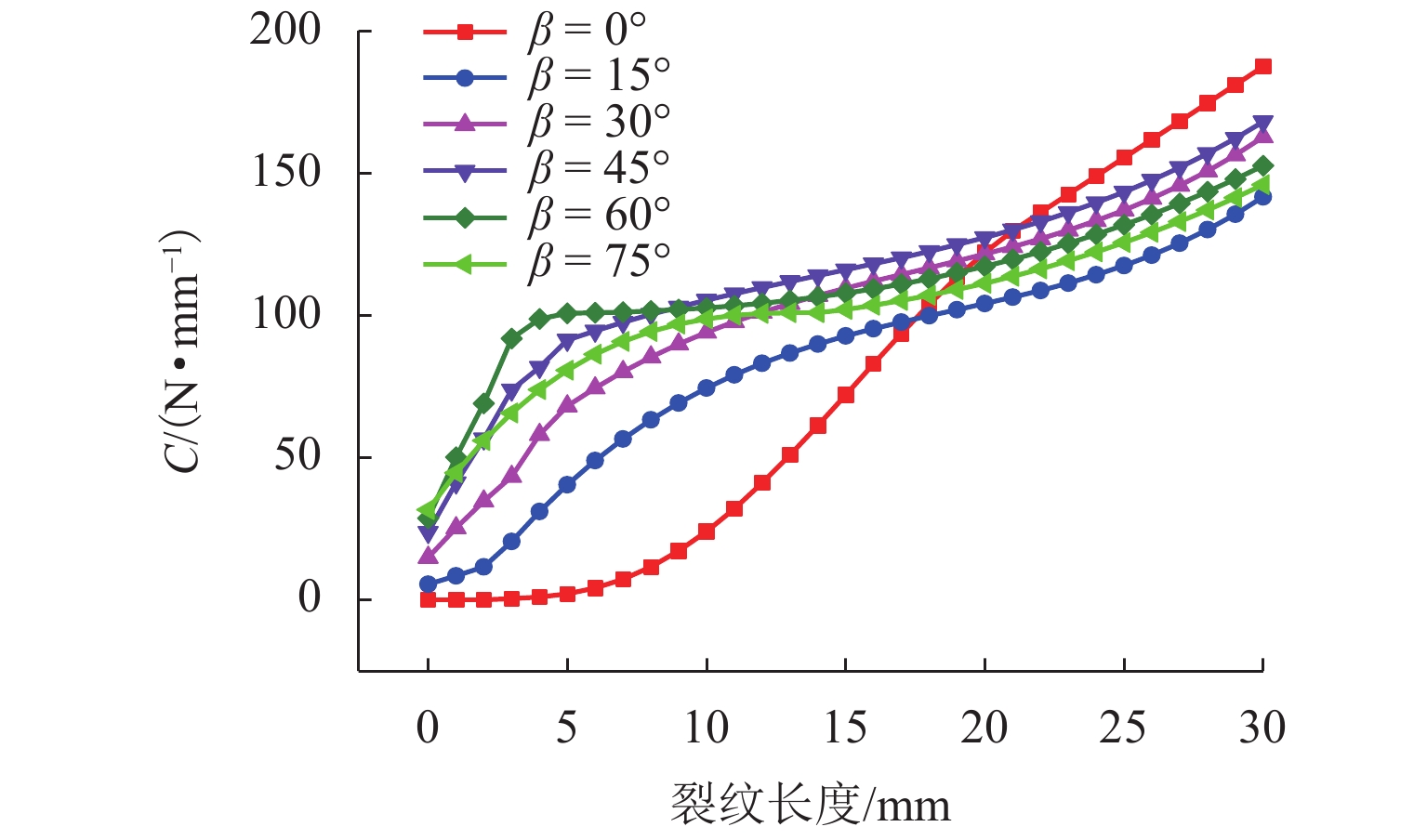
裂纹具有初始偏转角β时裂纹扩展过程如图10所示. 通过图10可以发现:当β=0° 时,裂纹扩展几乎不受骨料干涉的影响,当β≤45° 时,裂纹从起裂开始受到骨料的干涉作用,而向骨料的方向发生扩展;当β>45° 时(β=60°,75°),在纯拉伸荷载下,裂纹会以Ⅰ型裂纹方式进行扩展,之后受到骨料的干涉作用,而向骨料的方向发生扩展.
通过对裂尖构型力变化趋势(如图11所示)分析可知:当裂纹具有一定偏转角β时,裂纹裂尖构型力要明显超过无骨料干涉下的裂尖构型力,这说明在纯拉伸和在下,当裂纹具有初始偏转角β时,裂纹会优先起裂;当裂纹扩展具有一定初始偏转角β时,裂纹扩展结束时的裂尖构型力随着偏转角β的增大先增大后减小,说明裂纹“刺入”骨料时,骨料对裂纹扩展具有“止裂”效果,表现为材料抗裂性能的提高;当β=45° 时,裂尖构型力明显偏大,此时裂纹趋于非稳定状态,易发生扩展,骨料对裂纹扩展的抑制效果受β的影响.
3.2 非对称双骨料干涉作用
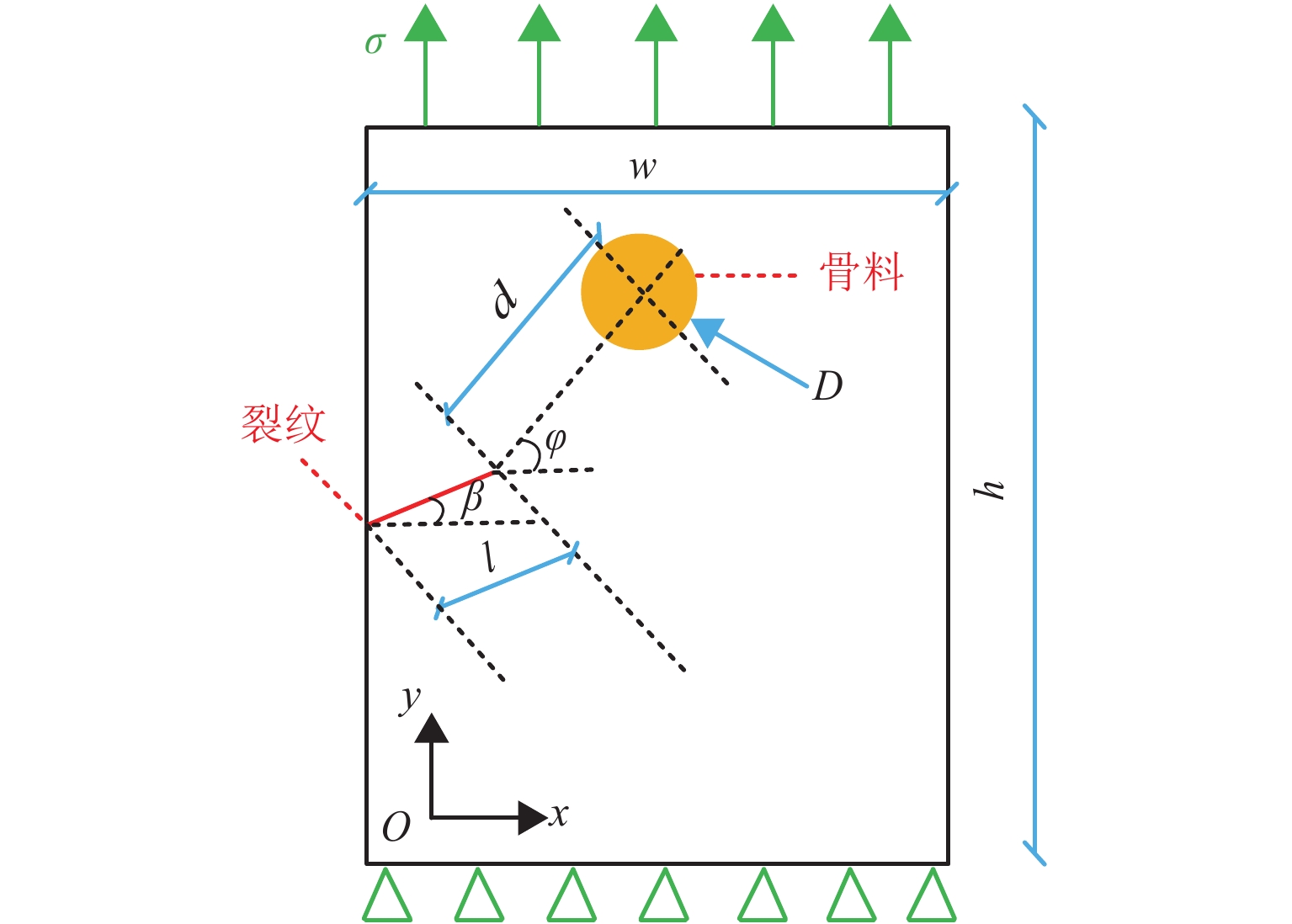
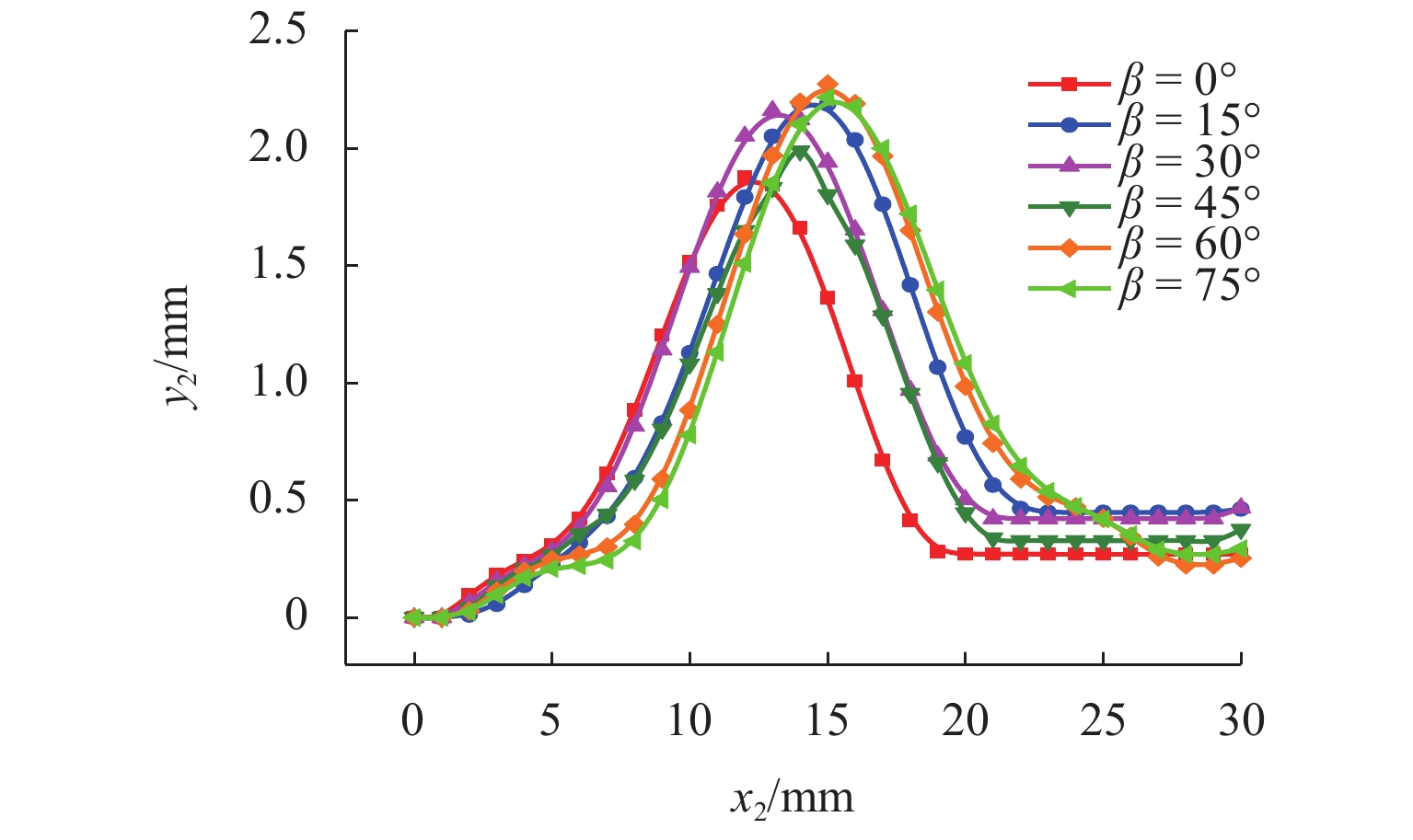
通过前文分析可知,当夹角γ>45° 时,骨料对裂纹扩展具有“止裂”效果,由于本节着重分析当裂纹具有初始裂纹偏转角时,非对称双骨料对裂纹扩展路径的影响,因此为避免裂纹与骨料发生聚合, 取γ=45°. 本次算例以仍以2.36 mm骨料为计算算例,且第一个骨料与初始裂尖的夹角φ取45°,d=8 mm,s=8 mm,β=0°,15°,30°,45°,60°,75°,如图12所示.
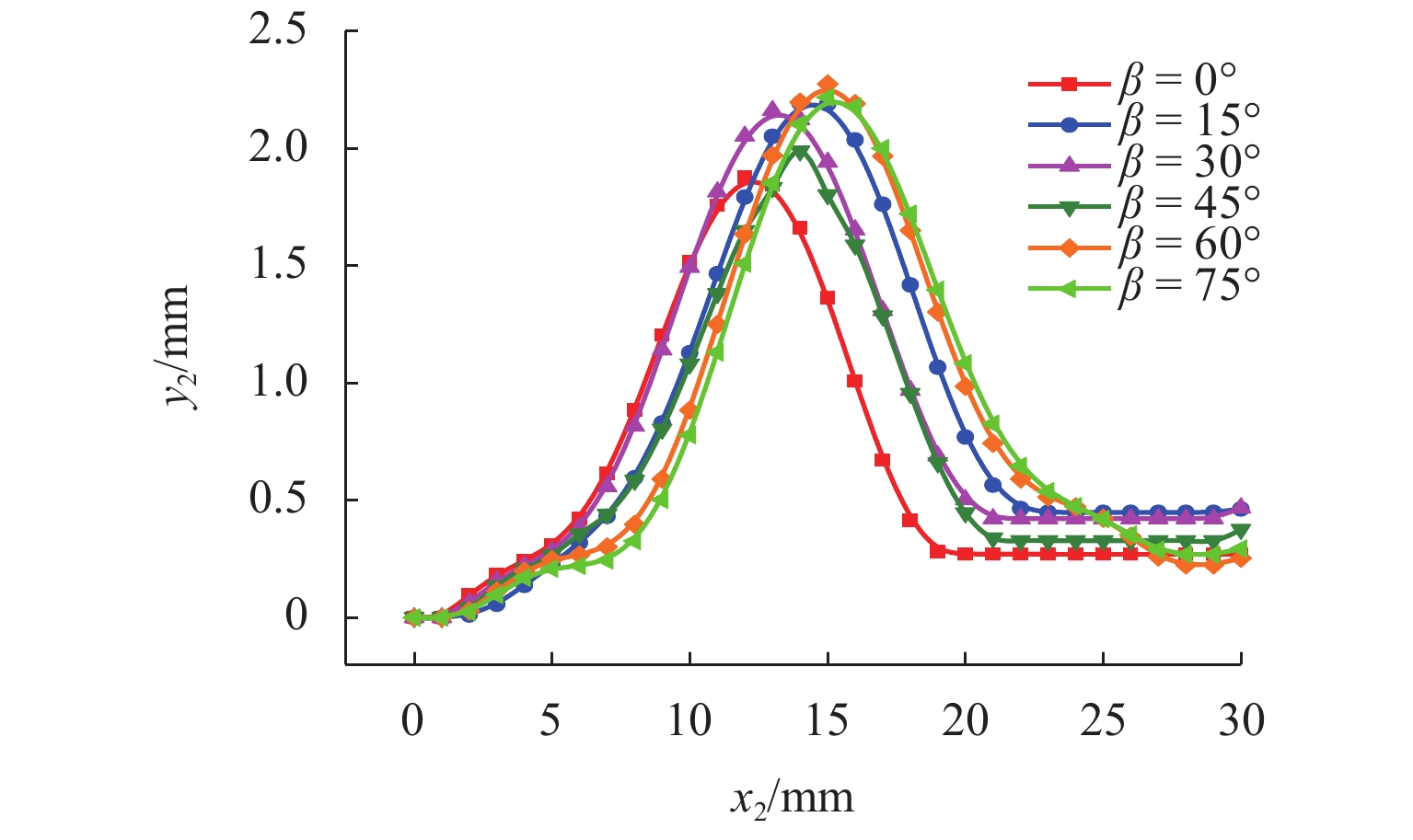
以初始裂纹尖端为坐标原点,通过提取裂纹扩展过程中裂尖坐标变化曲线,即裂纹扩展路径,分析不同条件下裂纹扩展的差异性,如图13所示.
由图13可知:在纯拉伸作用下,裂纹起裂之后以纯Ⅰ型裂纹形式发生扩展,随后在骨料的干涉作用下,裂纹扩展发生不同程度的偏离,并且裂纹在y轴方向上初始偏离量随着β的增大而逐渐减小,说明β向远离骨料的方向增大,造成裂尖与第一个骨料的在y轴方向上的相对位置增大,致使第一个骨料对裂纹的干涉作用逐渐减弱.
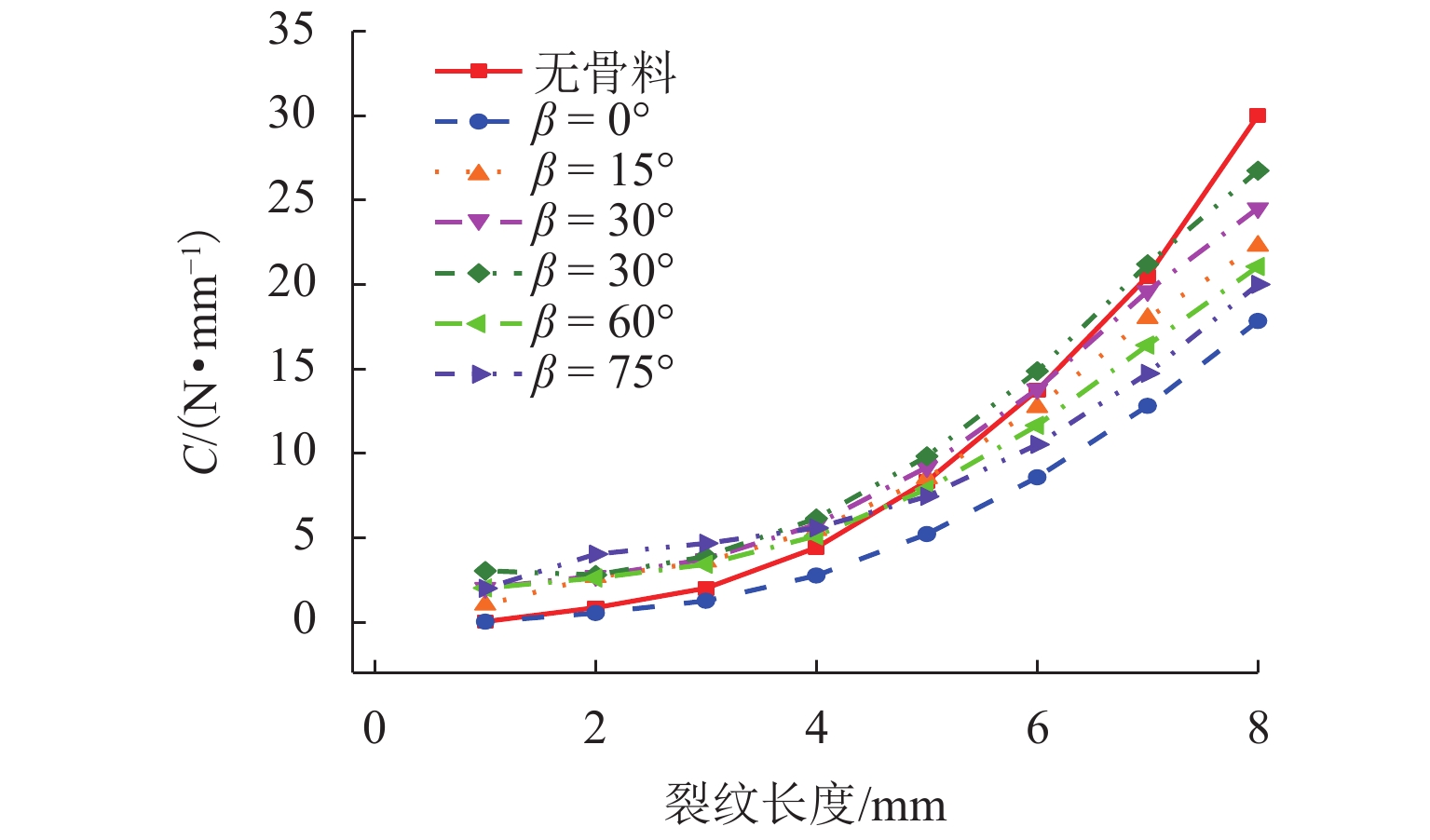
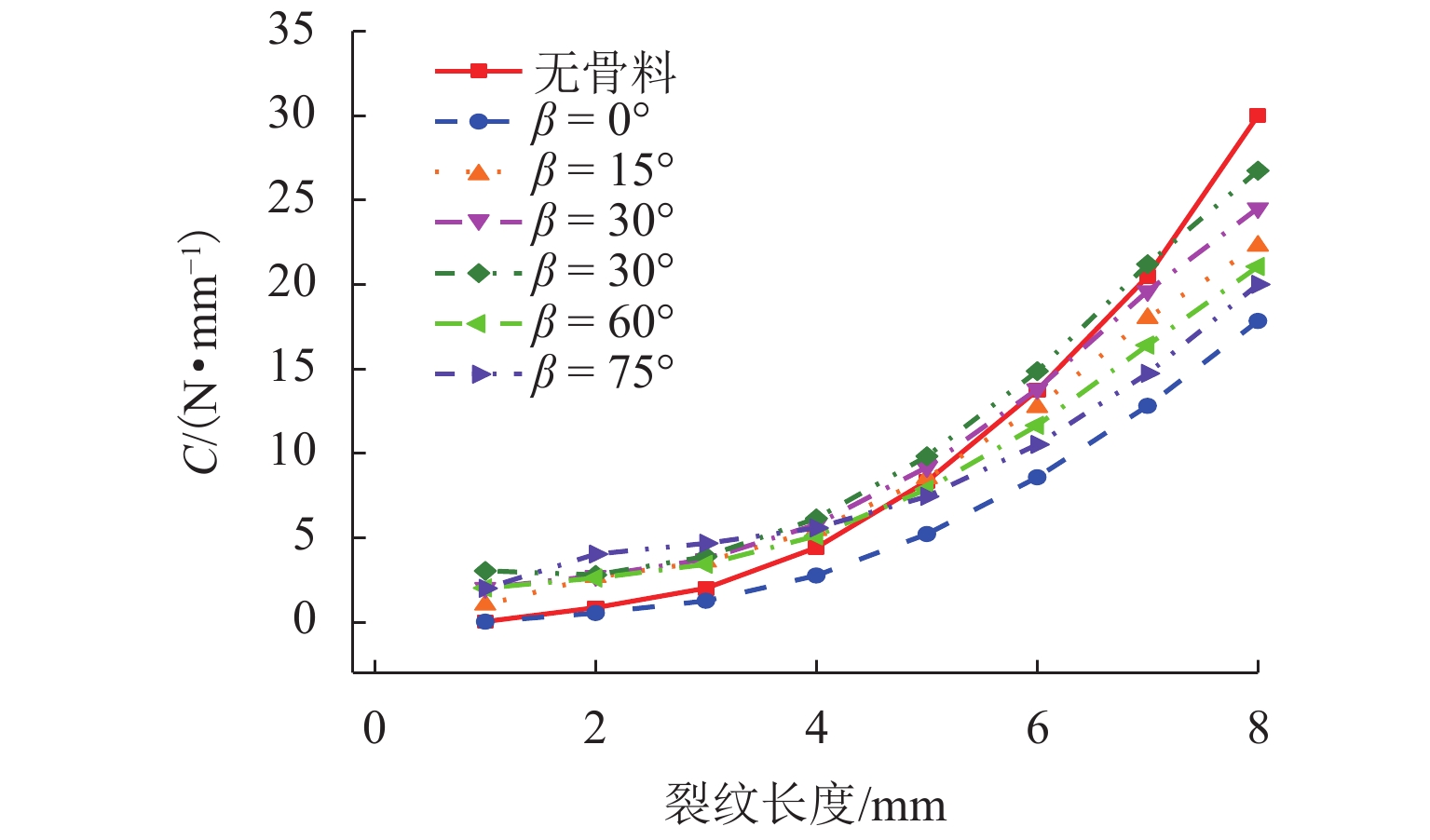
通过裂尖构型力随裂纹长度变化趋势(图14)分析可知:裂纹起裂时,裂尖构型力随着β的增加而逐渐增大,即裂纹容易发生扩展,进一步说明,第一个骨料对裂纹扩展的干涉作用逐渐减弱,致使裂纹趋于非稳定状态;随着裂纹扩展,裂尖构型力增长趋势表现出较大的差异性,即β=0° 的裂尖构型力迅速增长,而β≠0° 的裂尖构型力增长缓慢,说明在纯拉伸荷载下,第二个骨料对裂纹扩展逐渐起到明显的抑制作用.
然而,裂纹扩展结束时,β=0° 的裂尖构型力明显大于其余条件下的裂尖构型力,当β≠0° 时,裂尖构型力表现出一定差异性,双骨料能够在不同程度上抑制具有初始偏转角裂纹的扩展. 进一步通过比较β≠0° 时的裂尖构型力可以发现,随着β的增大,裂尖构型力先增大后减小,且在β=45° 时,裂尖构型力最大,说明此时双骨料对裂纹扩展的抑制效果较弱,裂纹趋于非稳定状态,易发生扩展,这意味着当裂纹与荷载作用方向呈45° 发生扩展时,由于骨料对裂纹扩展的抑制效果较弱,致使裂纹趋于非稳定状态,易发生扩展,形成宏观裂缝,从而使沥青混合料容易发生破坏.
4. 结 论
1) 在单骨料干涉作用下,骨料对裂纹扩展的干涉作用受φ的影响, 当φ≤45° 时,裂尖构型力一呈现非线性增长,表明骨料对裂纹扩展具有较强的干涉作用,沥青混合料具有较好的抗裂性能;随着夹角φ的增大,裂尖构型力逐渐减小,表明骨料的干涉作用逐渐减弱.
2) 在非对称双骨料干涉作用下,随着夹角γ的增大,裂尖构型力明显减小,说明骨料对裂纹扩展的抑制效果逐渐增强,当夹角γ>45° 时,由于骨料对裂纹扩展表现出“止裂”效果,使其裂尖构型力明显偏小.
3) 裂尖构型力随偏转角β的增大先增大后减小,当β=45° 时,裂尖构型力较大,骨料对裂纹扩展的干涉作用较弱,导致裂纹趋于非稳定状态,易发生失稳型扩展形成宏观裂缝,导致沥青混合料发生破坏.
-
表 1 AC-13沥青混凝土级配
Table 1. Gradation of AC-13 Asphalt Concrete
筛孔尺寸/mm 16.000 13.200 9.500 4.750 2.360 1.180 0.600 0.300 0.150 0.075 通过率/% 100.0 97.5 84.0 62.5 42.5 32.0 24.0 15.5 11.0 6.0 表 2 −20 ℃下AC-13级配沥青混凝土材料参数
Table 2. Parameters of AC-13 asphalt concrete at −20 ℃
材料相类 计算参数 数值 骨料 弹性模量 E/GPa 55.5 抗拉强度 σ/MPa 27.60 沥青混合料 抗拉强度 σ/MPa 3.55 断裂能/(J·m−2) 275 表 3 扩展有限元模型设置参数
Table 3. Parameters of extended finite element model
材料类型 参数类型 数值 沥青混合料 宽度 W/mm 30 长度 H/mm 40 裂纹 裂纹长度 l/mm 2 粗骨料 粒径 D/mm 2.36 d/mm 8 夹角\varphi /(°) 0、15、30、45、60、75、90 -
[1] ATKINSON C. The interaction between a crack and an inclusion[J]. International Journal of Engineering Science, 1972, 10(2): 127-136. doi: 10.1016/0020-7225(72)90011-0 [2] RUBINSTEIN A A. Macrocrack-microdefect interaction[J]. Journal of Applied Mechanics, 1986, 53(3): 505-510. doi: 10.1115/1.3171803 [3] ANIFANTIS N K. Crack surface interference: a finite element analysis[J]. Engineering Fracture Mechanics, 2001, 68(12): 1403-1415. doi: 10.1016/S0013-7944(01)00028-5 [4] MISHURIS G, MOVCHAN A, MOVCHAN N, et al. Interaction of an interfacial crack with linear small defects under out-of-plane shear loading[J]. Computational Materials Science, 2012, 52(1): 226-230. doi: 10.1016/j.commatsci.2011.01.023 [5] 毛成,邱延峻. 沥青混凝土复合型裂纹扩展行为数值模拟[J]. 公路交通科技,2006,23(10): 20-24. doi: 10.3969/j.issn.1002-0268.2006.10.005MAO Cheng, QIU Yanjun. Numerical simulation of compound crack propagation behavior of asphalt concrete[J]. Journal of Highway and Transportation Research and Development, 2006, 23(10): 20-24. doi: 10.3969/j.issn.1002-0268.2006.10.005 [6] 黄晓明,肖益民,张裕卿. 沥青混合料黏弹性裂纹扩展[J]. 东南大学学报(自然科学版),2009,39(3): 586-591.HUANG Xiaoming, XIAO Yimin, ZHANG Yuqing. Viscoelastic crack propagation in asphalt mixtures[J]. Journal of Southeast University (Natural Science Edition), 2009, 39(3): 586-591. [7] 郭荣鑫. 夹杂物干涉机制及其对材料细观损伤的影响研究[D]. 昆明: 昆明理工大学, 2007. [8] 束一秀,李亚智,尚海江,等. 基于XFEM研究含颗粒夹杂材料的疲劳裂纹行为[J]. 固体火箭技术,2016,39(4): 547-554.SHU Yixiu, LI Yazhi, SHANG Haijiang, et al. Fatigue crack behavior in metallic panels with inclusions using XFEM[J]. Journal of Solid Rocket Technology, 2016, 39(4): 547-554. [9] KIENZLER R, HERRMANN G. Fracture criteria based on local properties of the Eshelby tensor[J]. Mechanics Research Communications, 2002, 29(6): 521-527. doi: 10.1016/S0093-6413(02)00299-9 [10] GUO Y L, LI Q. Material configurational forces applied to mixed mode crack propagation[J]. Theoretical and Applied Fracture Mechanics, 2017, 89: 147-157. doi: 10.1016/j.tafmec.2017.02.006 [11] LARSSON R, FAGERSTRÖM M. A framework for fracture modelling based on the material forces concept with XFEM kinematics[J]. International Journal for Numerical Methods in Engineering, 2005, 62(13): 1763-1788. doi: 10.1002/nme.1246 [12] 贺启林. 基于J-积分和构型力理论的材料断裂行为研究[D]. 哈尔滨: 哈尔滨工业大学, 2010. [13] FAGERSTRÖM M, LARSSON R. Approaches to dynamic fracture modelling at finite deformations[J]. Journal of the Mechanics and Physics of Solids, 2008, 56(2): 613-639. doi: 10.1016/j.jmps.2007.05.001 [14] LI Z H, CHEN Q. Crack-inclusion interaction for mode Ⅰ crack analyzed by eshelby equivalent inclusion method[J]. International Journal Of Fracture, 2002, 118(1): 29-40. doi: 10.1023/A:1022652725943 [15] 古斌,郭宇立,李群. 基于构型力断裂准则的裂纹与夹杂干涉问题[J]. 力学学报,2017,49(6): 1312-1321. doi: 10.6052/0459-1879-17-209GU Bin, GUO Yuli, LI Qun. Crack interacting with an individual inclusion by the fracture criterion of configurational force[J]. Chinese Journal of Theoretical and Applied Mechanics, 2017, 49(6): 1312-1321. doi: 10.6052/0459-1879-17-209 [16] 于宁宇,李群. 基于数字散斑相关实验测量的材料构型力的计算方法[J]. 实验力学,2014,29(5): 579-588. doi: 10.7520/1001-4888-13-132YU Ningyu, LI Qun. On the algorithm of material configurational force based on digital image correlation measurement[J]. Journal of Experimental Mechanics, 2014, 29(5): 579-588. doi: 10.7520/1001-4888-13-132 [17] BELYTSCHKO T, BLACK T. Elastic crack growth in finite elements with minimal remeshing[J]. International Journal for Numerical Methods in Engineering, 1999, 45(5): 601-620. doi: 10.1002/(SICI)1097-0207(19990620)45:5<601::AID-NME598>3.0.CO;2-S [18] SUKUMAR N, CHOPP D L, MOëS N, et al. Modeling holes and inclusions by level sets in the extended finite-element method[J]. Computer Methods in Applied Mechanics and Engineering, 2001, 190(46/47): 6183-6200. [19] ESHELBY J D. The determination of the elastic field of an ellipsoidal inclusion, and related problems[J]. Proceedings of the Royal Society of London Series A: Mathematical and Physical Sciences, 1957, 241: 376-396. [20] 冷严. 季冻区高速铁路沥青混凝土强化基床表层材料制备技术与综合性能试验研究[D]. 成都: 西南交通大学, 2018. [21] YOU Z P, ADHIKARI S, DAI Q L. Three-dimensional discrete element models for asphalt mixtures[J]. Journal of Engineering Mechanics, 2008, 134(12): 1053-1063. doi: 10.1061/(ASCE)0733-9399(2008)134:12(1053) -





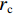
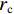

 下载:
下载:
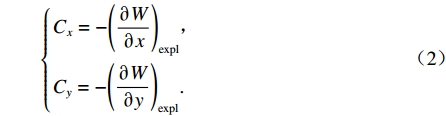


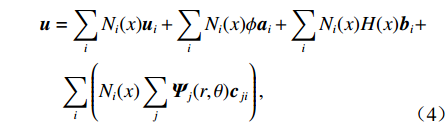

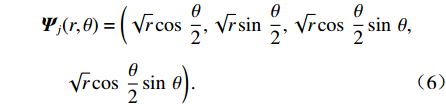
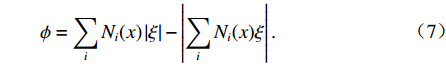
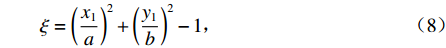







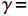







 下载:
下载: