Image Encryption Based on 2D Coupled Map Lattices
-
摘要:
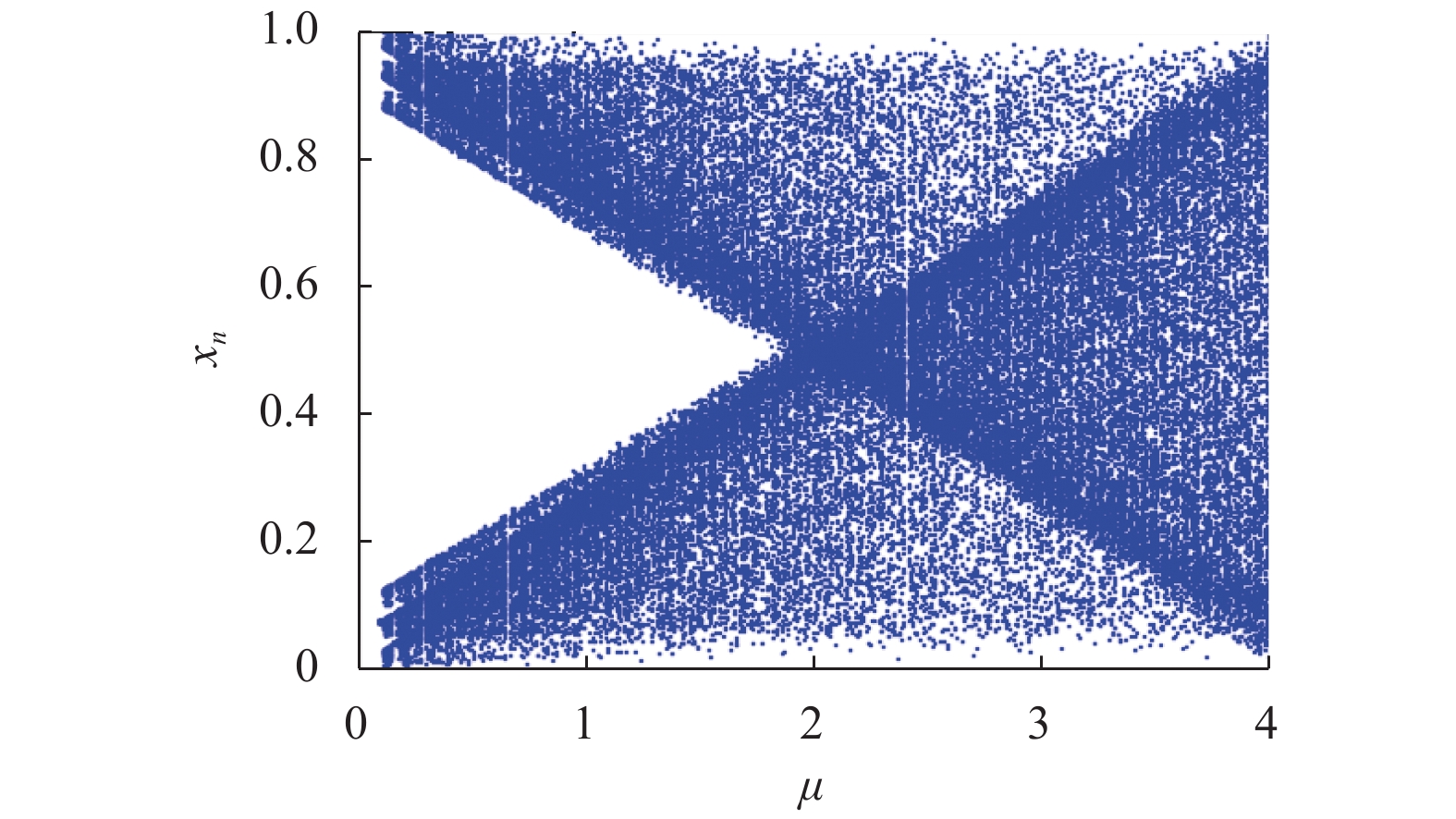
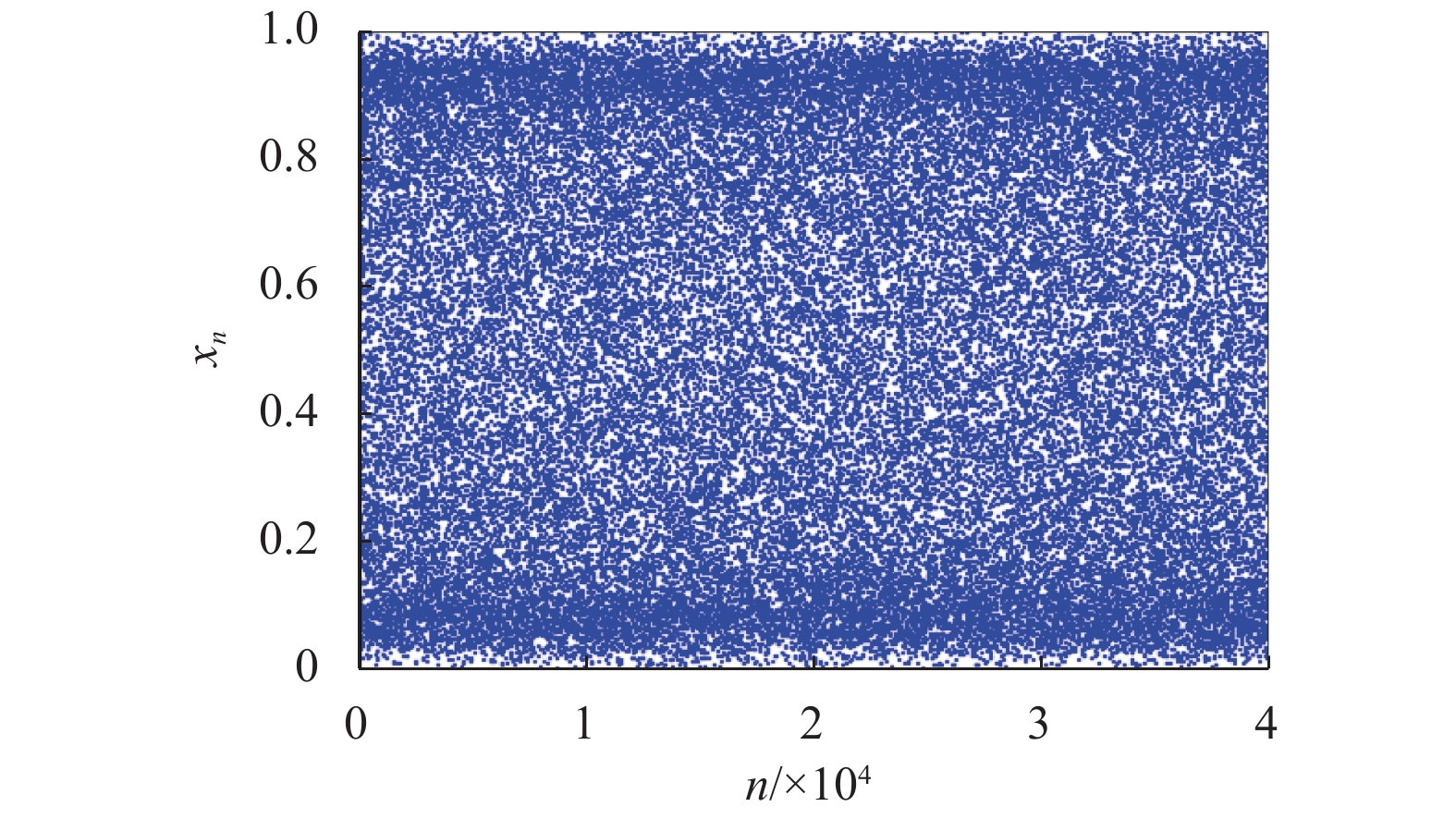
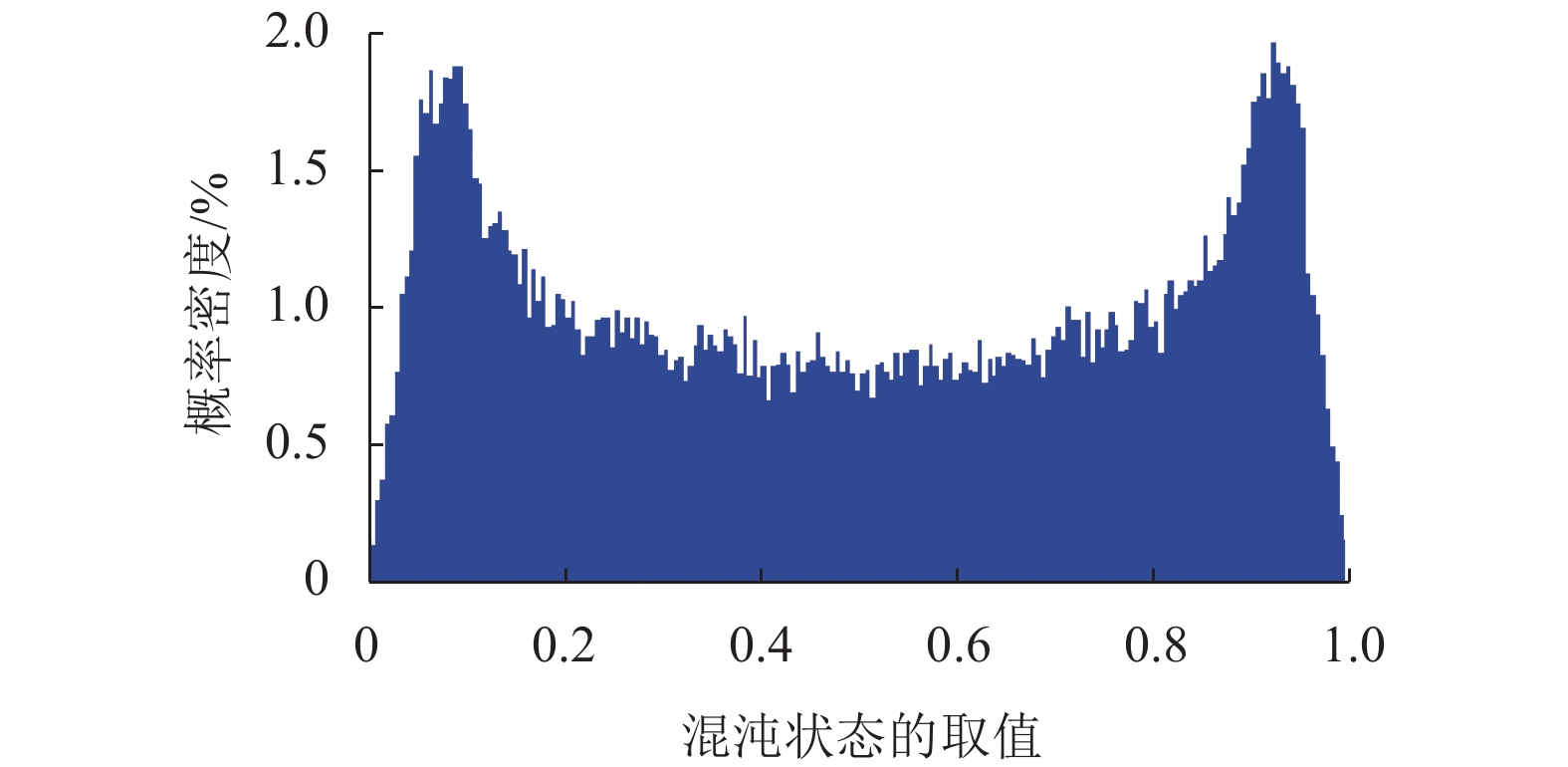
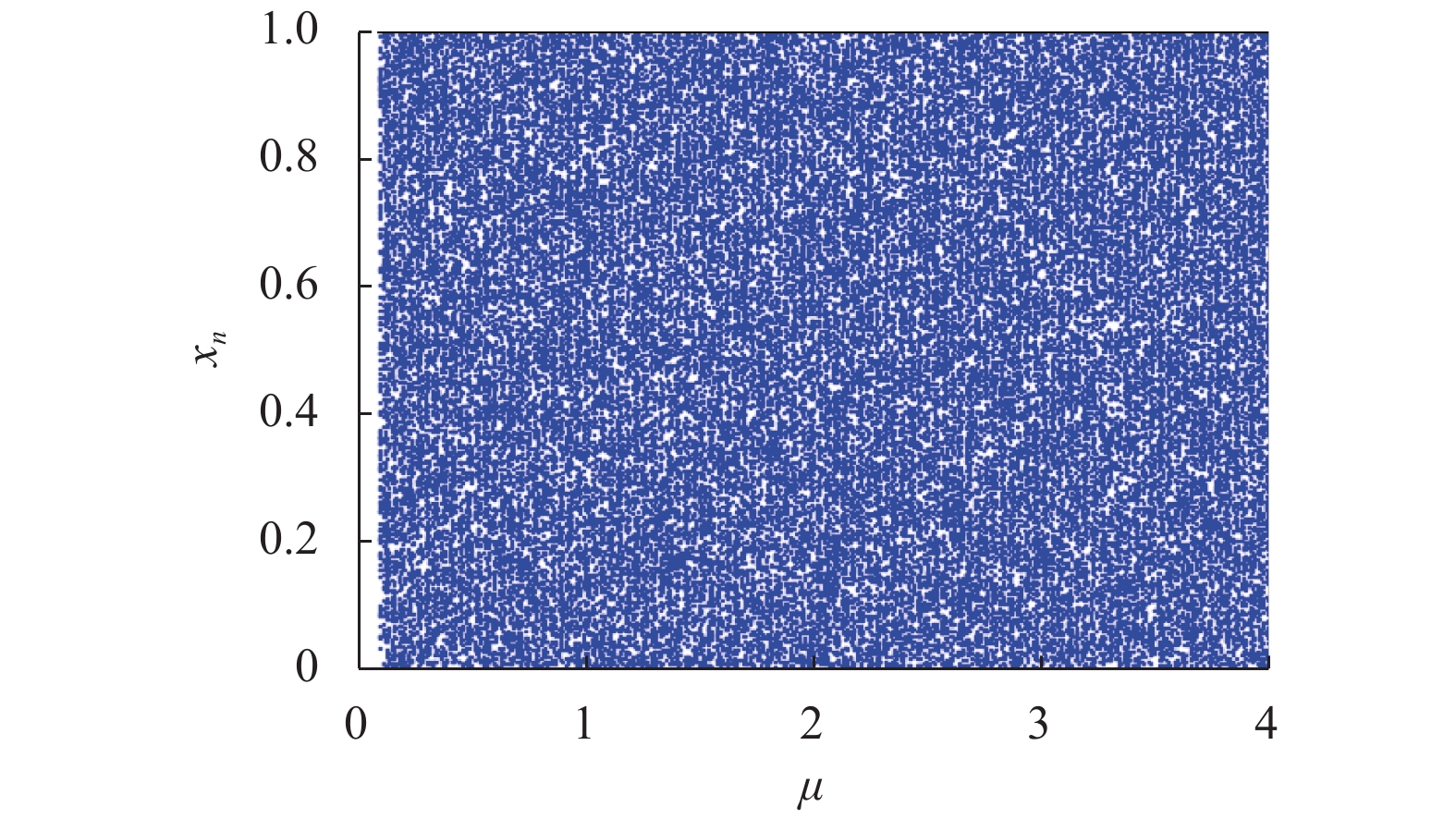
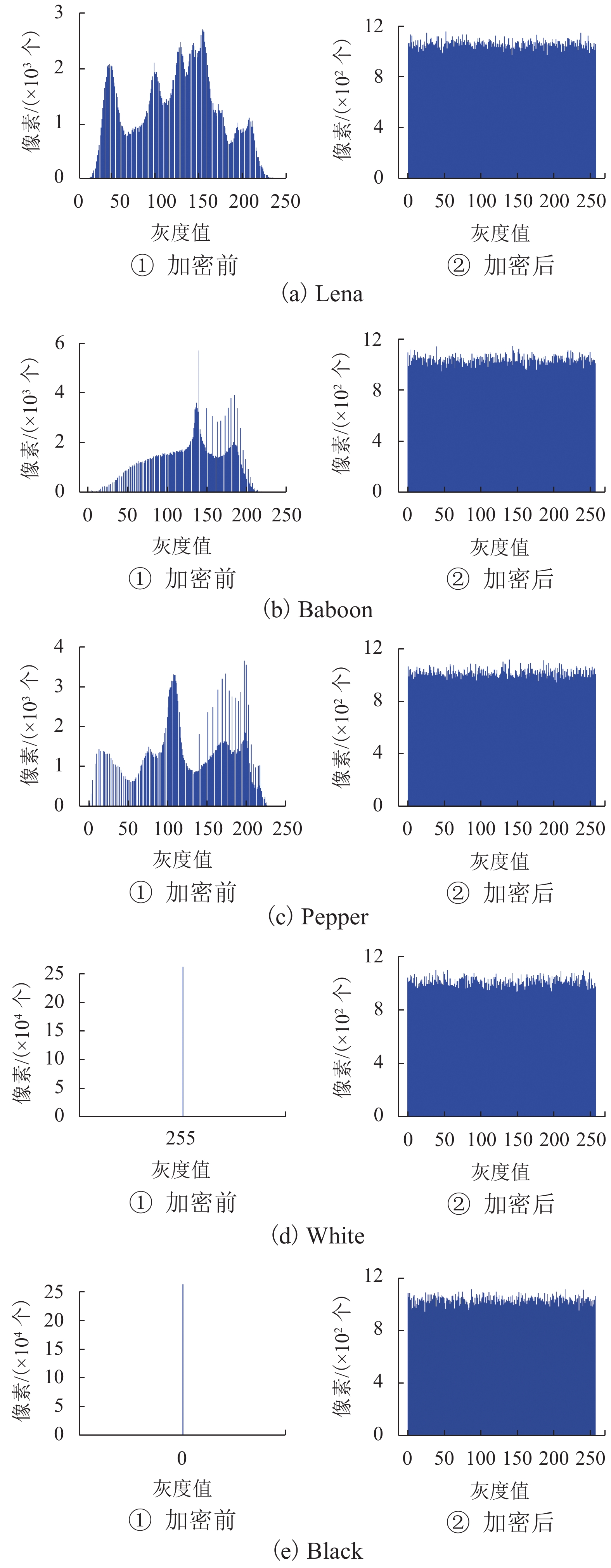
为了平衡混沌系统的复杂性和效率之间的关系，将分段Logistic映射（piecewise Logistic map，PLM）引入到二维耦合映像格子（2D coupled map lattices，2DCML）模型中. 采用暂态转换以使模型的输出序列服从均匀分布，进而得到T2DCML模型，基于此模型提出了一类图像加密算法. 在加密算法中，利用模型输出的伪随机序列构造两个初等变换矩阵，对图像进行置乱操作；然后再从模型中提取状态值的比特构造整数序列，对置乱后的图像进行扩散操作；经过若干轮的置乱与扩散操作，产生最后的加密图像. 仿真实验及性能分析表明：该算法的相关系数的绝对平均值为0.001 3，信息熵为7.999 3，像素变化率（number of pixel change rate，NPCR）和统一平均变化强度（unified average change intensity，UACI）分别为99.63%和33.60%，能够有效满足图像在网络中安全传输的需求.
-
关键词:
- 分段Logistic映射 /
- 二维耦合映像格子模型 /
- 混沌序列 /
- 图像加密
Abstract:Piecewise Logistic map (PLM) is introduced into 2D coupled map lattices (2DCML) model to face a tradeoff between complexity and efficiency of chaotic systems, and a transformation method based on transient status values is used to make the output sequence of the model obey a uniform distribution. Then, the T2DCML model is built. According to the T2DCML model, an image encryption algorithm is proposed, in which encryption algorithm, the pseudo-random sequence output by the model is used to construct two elementary transformation matrices and the image is scrambled by the matrices. Then, the bits of the state value are extracted from the model to construct an integer sequence and the scrambled image is diffused by the integer sequence. Finally, the encrypted image is produced by multi-rounds of confusion and diffusion. Simulation experiments and performance analysis show that the absolute average correlation coefficient of the algorithm is 0.001 3, the information entropy is 7.999 3, and the number of pixel change rate (NPCR) and the unified average change intensity (UACI) is 99.63% and 33.60%, respectively, revealing that the algorithm can effectively meet the needs of the safe transmission of images in the network.
-
Key words:
- piecewise Logistic map /
- 2D coupled map lattices /
- chaotic sequences /
- image encryption
-
城市地下管网作为城市基础设施建设中至关重要的一环，长期承受各种各样的上部荷载作用. 一旦管道发生破坏，轻则使路面破坏、影响交通，重则发生气体泄漏、引发火灾等次生灾害，威胁人身及财产安全[1]. 因此，研究埋地管道的力学与变形行为，以及如何对地下埋地管道进行防护尤为重要.
目前，对埋地管道的力学与变形性能研究主要从模型试验与数值模拟2个方面开展. 周恩全等[2]通过模型试验，证明在粉土中加入橡胶颗粒能够有效减小冲击荷载下路基表层沉降和管道应变及弯矩响应；张虎等[3]通过建立缩尺模型，研究冲击荷载作用下管道力学响应，并建立相应有限元模型进行数值模拟分析，验证数值模型的准确性；钟紫蓝等[4]通过三维有限元模拟对中密砂中不同深径比条件下不同破坏模式以及极限承载力的差异进行了讨论；肖成志等[5]通过有限元数值模拟与模型试验对比分析，验证了有限元数值方法的可靠性，并综合分析静载作用下各铺设因素对管道性能的影响.
近年来，土工格栅由于其高强度、低蠕变以及与土之间嵌锁作用好等优点，广泛应用于路堤、挡土墙和边坡当中，以提高其稳定性[6]. 目前，已有学者将其应用于埋地管道的防护：Moghaddas Tafreshi等[7]通过模型试验，研究土工格栅加筋对往复荷载作用下管道变形和土体表面沉降的影响，结果表明，土工格栅可以显著减少管道垂向直径的变化和土体表面沉降；肖成志等[8]通过模型试验展示土工格栅在埋设过程中各类参数对管道防护效果的影响；刘宽等[9]通过有限元软件ABAQUS，将管道与土工格栅按线弹性体考虑，分析静载作用下加载板宽度、土体内摩擦角以及管道埋深等因素对管道力学性能和变形特征的影响；肖成志等[10]通过有限元软件证明了增加土工格栅埋设长度对限制管道在循环荷载作用下的变形有显著作用.
数值模拟是试验研究的重要补充，而有限元软件只能模拟连续介质，不能真实体现出土体的非连续介质特性. 离散元方法考虑填料的大变形，也能模拟填料与加筋材料之间的咬合作用以及两者相对运动情况. 离散元模拟在岩土工程领域已经有广泛应用，如边坡工程，采矿、地下开挖和隧道工程，以及岩体动力学和隧道工程等方面[11].
目前，在竖向荷载作用下埋地管道力学性能及土工格栅加筋防护应用的数值模拟成果主要以有限元模拟结果为主，对系统内部土颗粒、土工格栅和管道三者之间的相互作用分析不够充分，且从细观层面对土、土工格栅和管道之间的相对运动与荷载传递规律的分析不够深入.
鉴于此，本文采用离散元软件，通过颗粒流程序（PFC）基本命令与FISH语言共同进行命令流的编制，建立复杂埋地管道数值仿真分析模型，研究竖向荷载作用下管道埋深与筋材埋深对管道-土体-土工格栅耦合系统力学性能的影响，分析其内部颗粒间接触力分布、颗粒移动规律等. 旨在通过阐述细观变化引起的宏观响应，增进对土工格栅加筋埋地管道力学与变形特性的认识.
1. 模型试验概述
1.1 试验系统
为分析竖向荷载作用下埋地管道的力学与变形特性，建立室内模型试验系统，如图1所示. 图中：D为管道模型外径，u为加筋后土工格栅埋深，H为竖向荷载作用下管道埋深，l为加筋后土工格栅长度. 模型箱内部尺寸长、宽、高分别为
1000 、580、700 mm，模型箱前后壁为钢化玻璃，以便对试验过程进行实时观察. 管道正上方采用一块厚度为10 mm，长、宽分别为500、200 mm的钢板施加竖向荷载. 为防止加载板在加载时产生竖向挠曲，在加载板上方中间局部焊接工字钢. 同时,在加载板上布设数显百分表以记录加载过程中的沉降数据，前后对称各布置一个并取其平均值作为结果以减小试验误差.1.2 试验材料参数
模型试验填料选择干净的粗砂，粒径较为均匀，其范围为0.5～2.0 mm，不均匀系数Cu=2.04，曲率系数Cc=1.01. 试验中控制填料的压实度为95%.
试验管道使用U-PVC塑料排水管，管道外径为110 mm，壁厚为3.2 mm.
试验中筋材为双向拉伸塑料土工格栅，其网孔尺寸为31 mm × 31 mm，抗拉强度为11.6 kN/m.
2. 离散元数值模型建立
2.1 模型细观参数的标定
为表征砂土、管道和土工格栅的物理力学特性，分别对3种材料的离散元细观参数进行标定.
2.1.1 砂土
采用线性接触模型以无黏结圆盘颗粒对砂土进行建模. 为提高计算机的计算效率，许多学者通过采用放大颗粒尺寸的方法以得到满意的结果[12]. 在本研究中，将砂土颗粒的粒径尺寸放大3倍. 离散元模型中砂土粒径级配曲线如图2所示. Wang等[13]提出一种将砂土的三维孔隙率向二维孔隙率转换的方法，经过抛物线方程转换与迭代计算，最终确定初始二维孔隙率为0.151.
对离散元砂土颗粒分别进行法向荷载50、100、200、300 kPa下的数值直剪试验，结果如图3所示. 可以看出：离散元模拟中，砂土颗粒直剪特性与室内试验中砂土直剪试验结果基本吻合.
2.1.2 管道
采用能够同时表征接触力与弯矩作用的平行黏结接触模型对管道进行模拟. D=110 mm，由直径相同的圆盘颗粒构成，颗粒直径为管道壁厚，即3.2 mm，依据塑料管材环刚度的测定试验建立管道数值压缩试验模型，对管道模型细观参数进行标定.
标定过程中上墙以恒定速率向下移动，对管道模型施加竖直方向荷载，并监测管道的压缩变形量以及管道模型对墙体的反作用力，获取压缩过程中管道模型的荷载-变形关系曲线，管道数值压缩模型与标定结果曲线如图4所示.
2.1.3 土工格栅
土工格栅离散元模型也采用平行黏结接触模型. 格栅模型由一串直径3 mm的圆盘颗粒组成，相当于将实际格栅厚度放大了3倍，以与砂土颗粒放大倍数相匹配. 在二维的PFC模拟（PFC2D）中，由于将三维网孔结构的土工格栅简化成二维离散元模型，为表征实际土工格栅与砂土颗粒之间的嵌锁咬合作用，参照Wang等[14]的方式在横肋对应位置处上下各设置3个突出的颗粒. 土工格栅拉伸数值模型及其标定后的对比结果如图5所示.
图 5 土工格栅数值模拟与试验结果对比Figure 5. Comparison between numerical simulation and test results of geogrid2.1.4 离散元模型参数取值
通过在标定过程中不断调整细观参数以及参考文献[15-16]中的取值，最终确定数值模拟中砂土、管道和土工格栅的细观参数，如表1所示. 表中：ρ1为颗粒相对密度，E1为平行黏结有效模量，S1为平行黏结法向强度，S2为平行黏结切向强度. 离散元标定结果与试验结果相匹配，表明PFC2D模拟中所采用的砂土、管道和土工格栅所输入的细观参数能够很好地反映砂土、管道和土工格栅的宏观力学特性.
表 1 离散元模型参数取值Table 1. Parameter used in discrete element model名称 接触模型 孔隙率 ρ1/
（kg·m−3）颗粒
直径/mm摩擦系数 有效模量/MPa 刚度比 E1/MPa 平行黏结刚度比 黏结激活间隙 S1/
（MPa·m−1）S2/
（MPa·m−1）砂土 线性 0.151 2650 1.5～6.0 4.00 20 1 管道 平行黏结 1500 3.2 0.25 8 2 1900.0 2 10−5 400.0 400.0 土工格栅 平行黏结 905 3.0 0.30 8 1 64.5 1 10−5 24.5 24.5 2.2 离散元数值模型建立
离散元数值模型依据试验中模型观测方向尺寸，即以实际模型长 × 高尺寸1∶1进行建模，模型长
1000 mm，高700 mm. 数值模型建立过程参照实际模型试验时的填筑步骤，总体自下而上生成. 施加竖向荷载前离散元数值模型示意如图6所示.
图 6 加筋埋地管道离散元数值模型示意Figure 6. Numerical discrete element model of reinforced buried pipelines本研究拟分析竖向荷载作用下管道埋深H、加筋后土工格栅埋深u对土体中含管道系统特性的影响，模拟方案如表2所示. 表中：B为加载板宽度. 在整体模型中，采用墙体命令wall作为加载板. 加载方式采用分级伺服加载，每级荷载10 kPa. 数值模拟与模型试验相同，均是以加载板底部砂土地基剪切破坏为加载的终止条件. 当模型达到破坏条件时，伺服加载会因达不到平衡条件进而持续进行加载，使砂土地基发生过量沉降导致其发生剪切破坏，此时视上一级荷载为极限荷载.
表 2 离散元数值模拟方案Table 2. Numerical simulation scheme of discrete element名称 H l u 未加筋 1.5D/2.0D/2.5D 土工格栅加筋 1.5D 5.0D 0.25B/0.50B/0.75B 3. 模拟结果与分析
3.1 数值模拟与模型试验结果对比分析
为验证离散元模拟结果的准确性，以模型试验中未加筋时管道埋深H=1.5D，2.0D，2.5D 3种工况为例，将不同H下加载板沉降随荷载变化曲线与各工况下模型系统极限承载力的离散元数值模拟结果和室内模型试验数据进行对比，结果如图7、8所示.
图 7 不同管道埋深时加载板荷载-沉降关系曲线Figure 7. Load-settlement curves of loading plate under different buried depths of pipelines
图 8 不同管道埋深时模型系统极限承载力结果对比Figure 8. Comparison of ultimate bearing capacity results of model system under different buried depths of pipelines从图7可以看出：离散元模拟得到的加载板沉降随荷载变化曲线与室内模型试验结果变化规律基本一致，即加载板沉降量随H的增加而增加；同级荷载下模拟数据与实测数据较为接近，但极限承载力在数值上有较大差异.
由图8可知：虽然模型的极限承载力数值模拟结果与实测值大小存在差异，但是具有相同的变化趋势，即随着H的增加，模型的极限承载力增加，表明增加管道上方的填土厚度有助于提高模型的极限承载力.
通过图7和图8的对比结果可知，离散元数值模拟与实际模型箱试验的结果吻合较好，验证了本离散元模型的可行性以及模型中材料参数取值的合理性. 因此，本文将基于PFC2D离散元方法对不同管道埋深与单层加筋不同土工格栅埋深时管道系统的内部细观力学特性进行深入研究.
3.2 未加筋时管道埋深的影响
通过调整管道上方填土的高度，对未加筋时不同管道埋深即H/D=1.5，2.0，2.5 3种工况条件下模型内部颗粒间接触力、颗粒位移和管道垂直径向变形等进行分析.
模型内部荷载传递是通过颗粒间的接触力链传递的，接触力链的分布特征实质是土中荷载传递特征的体现. 图9为竖向荷载p=70 kPa作用下不同工况土体内部颗粒接触力链分布对比. 不同工况中线条均按同一比例绘制，力链越粗表示接触力越大. 需要说明的是，p=70 kPa为数值模拟中管道埋深H=1.5D时所对应的极限荷载. 由图9可知，加载板荷载在土体中以一定的角度扩散，并且在向下传递的过程中逐渐消散；整体来看，当H不同时力链分布均呈现出中间大、两侧小的分布规律；随着H的增大，荷载的传递路径增加，管道上方土颗粒对荷载的消散作用增强，所以管道受到荷载的影响作用减弱.
图 9 不同管道埋深时模型内部接触力链分布Figure 9. Distribution of contact force chain in model under different buried depths of pipelines图10为不同工况模型内部颗粒位移对比，位移值在同一等分范围内颗粒用同一种颜色进行标记. 从图中可以看出：不同管道埋深条件下各工况颗粒位移具有相似的变形模式，即颗粒最大位移均发生在加载板下方；加载板下方土体明显形成一个三角压密区，压密区向两侧挤压，土中产生塑性区，并贯通至地面，形成连续滑动面，此时土体从加载板两侧被挤出，形成竖向隆起. 塑性贯通区下方土颗粒位移等值线向下发展，经过管道时发生明显变化，管道下方土颗粒位移值远小于两侧同高度土颗粒，证明管道承受了主要的竖向荷载作用；在相同竖向荷载作用下，随着管道埋深的增加，加载板下方土体颗粒产生的沉降值更大，同时由于管道上方可以产生压缩变形的土体厚度增加，加载板的沉降量更大；管道埋深越浅时，加载板下方的三角形压密区距离管道位置更近，大变形量的上覆土对管道变形产生更大的影响；由于管道距土表距离减小，管道对上方土体产生更大的反作用，导致相同荷载作用下管道埋深越小时加载板下方塑性区更大，加载板两侧产生的土体表面隆起更大，也就是说管道的埋深越小，其上方填土更容易发生剪切破坏.
图 10 不同管道埋深时模型内部颗粒位移云图Figure 10. Particle displacement cloud diagram in model under different buried depths of pipelines图11为不同管道埋深下管道垂直径向变形量随荷载变化曲线对比. 可以看出，在相同荷载作用下，管道垂直径向变形量随着管道埋深的增加而减小. 以管道埋深H=1.5D的极限荷载为例进行分析，在70 kPa竖向荷载作用下，当H从1.5D增加到2.0D时，管道垂直径向变形量减小了12%，当H增加到2.5D时，与H=1.5D相比，管道垂直径向变形量减少了17%，这些结果与前文得到的结论基本一致,并且肖成志等[5]也曾在有限元模拟中得到类似的结果.
图 11 不同管道埋深时管道垂直径向变形量随荷载变化Figure 11. Variation of vertical radial deformation of pipelines with load under different buried depths of pipelines3.3 土工格栅埋深的影响
通过对未加筋时不同工况的对比可以发现，在管道埋深越小时，上覆土体越容易发生剪切破坏，承载力低且管道的垂直径向变形量大，因此，本节针对H=1.5D的工况分别进行单层土工格栅加筋，对其荷载-沉降曲线、模型内部颗粒间接触力、颗粒位移和管道垂直径向变形等进行分析，u分别取0.25B、0.50B和0.75B.
图12为管道埋深H=1.5D时管道上方不同土工格栅埋深条件下加载板荷载-沉降关系曲线. 从图中可以看出：在对管道上方进行加筋后，与未加筋时相比，在相同荷载作用下加载板沉降量均不同程度减小，而且加载板沉降量随着土工格栅埋深的减小而减小；土工格栅加筋后模型的极限承载力均增加，当u=0.25B时，与未加筋时相比，其极限承载力从70 kPa提高到了110 kPa，提高了约57.2%；当土工格栅的埋深增加后，土工格栅对模型承载力的提高作用逐渐减弱；加筋后各工况加载板沉降量与极限承载力变化趋势与肖成志等[17]在模型试验中得到的结果相同.
图 12 不同土工格栅埋深时加载板荷载-沉降关系曲线Figure 12. Load-settlement curves of loading plate under different buried depths of geogrid图13为土工格栅加筋时不同筋材埋深下管道垂直径向变形量随荷载变化对比. 从图中可以看出：当u=0.75B时，加载过程中管道的垂直径向变形量与未加筋时相差不大；当u=0.25B时，管道变形量减小明显，与u=0.75B相比，管道垂直径向变形量减小了约27.9%. 这表明土工格栅距离土体表面越近即土工格栅埋深越浅时，其加筋对管道的防护作用越好. 但需要注意的是，为保证土工格栅可以充分发挥其嵌锁作用，土工格栅上方需要留有一定厚度的土体，即土工格栅的埋深不能过浅.
图 13 不同土工格栅埋深时管道垂直径向变形随荷载变化Figure 13. Variation of vertical radial deformation of pipelines with load under different buried depths of geogrid图14为土工格栅加筋后竖向荷载p=70 kPa作用下不同工况模型内部土体颗粒沉降位移云图. 可见：1） 当u=0.25B时，与未加筋时相比，加载板两侧的隆起现象得到明显改善；颗粒向下沉降变形的方式主体上变成了由土工格栅埋设位置处向下发展，土体的整体性增强，土体塑性区变形受到一定的限制. 2） 当u=0.50B时，其加筋位置恰好位于未加筋时土体剪切破坏区域中部，与未加筋时工况相比，土体的变形模式变化不大，但此时加载板与土工格栅之间土体已经接近塑性破坏的状态. 由于土工格栅与土的相互作用，在后续施加下一级荷载后，土体并未出现破坏，这也体现了土工格栅的加筋效果. 同时，朗肯被动区滑动面与水平面的夹角逐渐减小，并且左右两侧被动区滑裂面由加载板两端点下方向加载板内部分延伸. 3） 当u=0.75B时，加筋使土体的滑动面向土表移动，且滑动区域范围减小. 4） 在产生沉降的土体中部进行土工格栅加筋时，土体沉降变形的扩散范围有一定程度的改善. 但是土工格栅距离上部加载板距离太远，不能充分发挥加筋作用.
图 14 不同土工格栅埋深时模型内部颗粒位移云图Figure 14. Particle displacement cloud diagram in model under different buried depths of geogrid图15为加筋后竖向荷载p=70 kPa作用下不同工况土体内部力链图分布情况. 不同工况中线条均按同一比例绘制，黑色代表压力，绿色代表拉力，力链越粗表示接触力越大. 从图中可以看出，由于土工格栅与土相互作用，土压力经过土工格栅后传递到管道与未加筋时分布更加均匀. 对比图15（b）～（d）可以发现：土工格栅位置越靠上，其上方土体间力链的扩散角越小；竖向荷载附加应力均匀传递至土工格栅位置后在土工格栅处产生应力扩散，使土工格栅下部土压力分布更加均匀；当u=0.50B时（图15（c）），加载板下方被动区滑裂面由加载板两侧端点向加载板内侧延伸，导致该位置处颗粒发生较大位移，此处力链相较于u=0.25B和u=0.75B明显减小；对于土工格栅拉力而言，土工格栅的最大拉力出现在加载板下方；竖向荷载附加应力扩散现象随着填土深度的增加愈加明显，导致土工格栅埋深大时拉力作用范围增大，力链也更粗；当u=0.75B时，其对上方土压力分布的改善不明显.
图 15 不同土工格栅埋深时模型内部接触力链分布Figure 15. Distribution of contact force chain in model under different buried depths of geogrid图16为竖向荷载p=70 kPa作用下各工况土工格栅拉力定量分布情况. 与图14进行对比分析可以发现：土工格栅拉力产生骤然变化的位置主要处于模型内部颗粒位移变化较大处. 当u=0.25B时，其距离填土顶部加载板较近，拉力在加载板端点下方突增；随着u的增加，土工格栅拉力最大值逐渐趋近于加载板下方中心；随着距管道中心距离的逐渐增大，土工格栅拉力呈现逐渐减小的变化规律；由于荷载在土体中的扩散效应，加载板下方外侧土工格栅拉力随着筋材埋深的增加而增大.
图 16 管道上方土工格栅拉力定量分布对比Figure 16. Quantitative distribution comparison of geogrid tension above pipelines4. 结 论
本文基于室内模型试验，建立离散元数值模型，对细观参数进行标定，验证了数值模拟结果的合理性，从细观层面探究管道埋深和土工格栅埋深对管道系统性能的影响，得出以下结论：
1） 模型系统极限承载力随着管道埋深的增大而增大；相同竖向荷载作用下，管道埋深越大，加载板竖向沉降量越大，管道垂直径向变形量越小.
2） 对管道上方进行单层土工格栅加筋后，模型系统极限承载力均不同程度增大，当u=0.25B时极限承载力最大. 随着土工格栅埋深逐渐增大，加筋效果逐渐减弱，相同竖向荷载作用下加载板沉降量和管道垂直径向变形量均增加.
3） 接触力链分布图与颗粒位移分布图可从细观角度更好地理解管道周围的荷载传递规律与土体变形特性. 未加筋时土体内部土压力分布呈现出中间大、两侧小的分布规律. 相同竖向荷载作用下管道埋深越大，受竖向荷载附加应力的影响越小；管道埋深越小，管道上方土体塑性贯通区越大.
4） 当u=0.25B时，其加筋作用很大程度上减小了管道上方土体的潜在塑性破坏区，并使土体内部土压力分布更加均匀；当土工格栅加筋位置在土体塑性破坏区下方时，土工格栅加筋对土体破坏的模式影响较小.
-
表 1 NIST套件的测试结果
Table 1. Test results of NIST suites
测试指标 P 值 通过率 结果 Frequency 0.719 747 0.993 通过 BlockFrequency 0.996 335 0.988 通过 CumulativeSums* 0.817 162 0.995 通过 Runs 0.765 632 0.988 通过 LongestRun 0.071 620 0.987 通过 Rank 0.179 584 0.990 通过 FFT 0.492 436 0.992 通过 NonOverlappingTemplate* 0.496 164 0.990 通过 OverlappingTemplate 0.209 948 0.983 通过 Universal 0.755 819 0.989 通过 ApproximateEntropy 0.075 719 0.986 通过 RandomExcursions* 0.627 887 0.989 通过 RandomExcursionsVariant* 0.540 402 0.992 通过 Serial* 0.676 598 0.986 通过 LinearComplexity 0.096 000 0.997 通过 
下载: 导出CSV
表 2 加密前后相邻像素间的相关系数
Table 2. Correlation coefficients between adjacent pixels
图像 水平方向 垂直方向 对角方向 Lena 明文 0.975 1 0.983 1 0.958 2 Lena 密文 0.001 2 0.000 6 0.001 6 Baboon 明文 0.888 0 0.746 8 0.716 0 Baboon 密文 0.001 1 0.000 4 0.003 3 Pepper 明文 0.977 4 0.975 8 0.962 7 Pepper 密文 0.000 8 0.002 6 0.001 2 White 明文 1.000 0 1.000 0 1.000 0 White 密文 −0.001 4 −0.000 1 0.002 9 Black 明文 1.000 0 1.000 0 1.000 0 Black 密文 0.003 0 −0.000 5 −0.002 2
下载: 导出CSV
表 3 加密前后图像的信息熵
Table 3. Information entropies of images
图像 明文信息熵 密文信息熵 Lena 7.445 5 7.999 3 Baboon 7.222 2 7.999 2 Pepper 7.364 4 7.999 3 White 0 7.999 3 Black 0 7.999 2
下载: 导出CSV
表 4 密文图像的差异
Table 4. Differences between ciphertext images
% 图像 测试 1 测试 2 测试 3 测试 4 Lena 99.592 99.614 99.598 99.602 Baboon 99.619 99.599 99.592 99.598 Pepper 99.621 99.622 99.615 99.619 White 99.623 99.618 99.613 99.617 Black 99.607 99.611 99.617 99.579
下载: 导出CSV
表 5 密文图像的NPCR和UACI
Table 5. NPCR and UACI of ciphertext images
% 图像 NPCR UACI Lena 99.59 33.44 Baboon 99.64 33.50 Pepper 99.60 33.50 White 99.59 33.53 Black 99.60 33.49
下载: 导出CSV
表 6 算法性能对比
Table 6. Comparison of algorithm performance
加密算法 相关系数 信息熵 NPCR/% UACI/% 水平 垂直 对角 绝对平均值 本文算法 −0.002 5 −0.000 2 0.001 1 0.001 3 7.999 3 99.63 33.60 文献[4] −0.022 3 −0.008 4 −0.008 6 0.013 1 7.997 4 99.61 33.46 文献[5] −0.038 1 −0.029 1 0.002 7 0.023 3 7.999 2 99.61 33.45 文献[6] 0.069 3 0.061 0 −0.024 2 0.051 5 7.999 1 99.57 33.41 文献[7] 0.001 4 0.003 8 0.001 1 0.002 1 7.999 3 99.59 文献[8] −0.023 0 0.001 9 −0.003 4 0.009 4 7.969 6 99.62 33.51 文献[9] −0.014 4 −0.003 4 0.010 7 0.009 5 7.997 0 99.60 32.91 文献[10] 0.016 3 −0.002 9 0.030 9 0.016 7 7.999 3 99.60 33.45
下载: 导出CSV
-
[1] GARCIA-BOSQUE M, DÍEZ-SEÑORANS G, PÉREZ-RESA A, et al. A 1 gbps chaos-based stream cipher implemented in 0.18 μm CMOS technology[J]. Electronics, 2019, 8(6): 623. doi: 10.3390/electronics8060623 [2] TEH J S, TAN K J, ALAWIDA M. A chaos-based keyed hash function based on fixed point representation[J]. Cluster Computing, 2019, 22(2): 649-660. doi: 10.1007/s10586-018-2870-z [3] ÖZKAYNAK F. Construction of robust substitution boxes based on chaotic systems[J]. Neural Computing and Applications, 2019, 31(8): 3317-3326. doi: 10.1007/s00521-017-3287-y [4] ZHANG Y. The fast image encryption algorithm based on lifting scheme and chaos[J]. Information Sciences, 2020, 520: 177-194. doi: 10.1016/j.ins.2020.02.012 [5] ZHOU Minjun, WANG Chunhua. A novel image encryption scheme based on conservative hyperchaotic system and closed-loop diffusion between blocks[J]. Signal Processing, 2020, 171: 107484.1-107484.14. [6] FARAH M A B, GUESMI R, KACHOURI A, et al. A novel chaos based optical image encryption using fractional Fourier transform and DNA sequence operation[J]. Optics & Laser Technology, 2020, 121: 105777.1-105777.9. [7] 张健,霍达. 基于混沌系统和DNA编码的量子图像加密算法[J]. 西南交通大学学报,2018,53(6): 1142-1149. doi: 10.3969/j.issn.0258-2724.2018.06.008ZHANG Jian, HUO Da. Quantum image encryption algorithm based on chaotic system and DNA coding[J]. Journal of Southwest Jiaotong University, 2018, 53(6): 1142-1149. doi: 10.3969/j.issn.0258-2724.2018.06.008 [8] XU Lu, ZHI Li, JIAN Li, et al. A novel bit-level image encryption algorithm based on chaotic maps[J]. Optics and Lasers in Engineering, 2016, 78: 17-25. doi: 10.1016/j.optlaseng.2015.09.007 [9] YE Guodong. A block image encryption algorithm based on wave transmission and chaotic systems[J]. Nonlinear Dynamics, 2014, 75(3): 417-427. doi: 10.1007/s11071-013-1074-6 [10] HUANG Xiaoling, YE Guodong. An image encryption algorithm based on hyper-chaos and DNA sequence[J]. Multimedia Tools and Applications, 2014, 72(1): 57-70. doi: 10.1007/s11042-012-1331-6 [11] NEPOMUCENO E G, NARDO L G, ARIAS-GARCIA J, et al. Image encryption based on the pseudo-orbits from 1D chaotic map[J]. Chaos:an Interdisciplinary Journal of Nonlinear Science, 2019, 29(6): 061101.1-061101.8. doi: 10.1063/1.5099261 [12] ALAWIDA M, TEH J S, SAMSUDIN A, et al. An image encryption scheme based on hybridizing digital chaos and finite state machine[J]. Signal Processing, 2019, 164: 249-266. doi: 10.1016/j.sigpro.2019.06.013 [13] GOPALAKRISHNAN T, RAMAKRISHNAN S. Image encryption using hyper-chaotic map for permutation and diffusion by multiple hyper-chaotic maps[J]. Wireless Personal Communications, 2019, 109(1): 437-454. doi: 10.1007/s11277-019-06573-x [14] 张健,霍达. 基于混沌系统的量子彩色图像加密算法[J]. 西南交通大学学报,2019,54(2): 421-427.ZHANG Jian, HUO Da. Quantum colour image encryption algorithm based on chaotic systems[J]. Journal of Southwest Jiaotong University, 2019, 54(2): 421-427. [15] WANG Xingyuan, ZHAO Hongyu, HOU Yutao, et al. Chaotic image encryption algorithm based on pseudo-random bit sequence and DNA plane[J]. Modern Physics Letters B, 2019, 33(22): 1950263.1-1950263.24. doi: 10.1142/S0217984919502634 [16] GAYATHRI J, SUBASHINI S. A spatiotemporal chaotic image encryption scheme based on self adaptive model and dynamic keystream fetching technique[J]. Multimedia Tools and Applications, 2018, 77(19): 24751-24787. doi: 10.1007/s11042-018-5675-4 [17] WEN Heping, YU Simin, LÜ Jinhu. Breaking an image encryption algorithm based on DAN encoding and spatiotemporal chaos[J]. Entropy, 2019, 21(3): 246. doi: 10.3390/e21030246 [18] WANG Yong, LIU Zhaolong, MA Jianbin, et al. A pseudorandom number generator based on piecewise logistic map[J]. Nonlinear Dynamics, 2016, 83(4): 2373-2391. doi: 10.1007/s11071-015-2488-0 [19] 王永,赵毅,GAO J,等. 基于分段Logistic映射的二维耦合映像格子模型的密码学相关特性分析[J]. 电子学报,2019,47(3): 657-663.WANG Yong, ZHAO Yi, GAO J, et al. Cryptographic feature analysis on 2D coupled map lattices based on piecewise logistic map[J]. Acta Electronica Sinica, 2019, 47(3): 657-663. [20] 李佩玥,石俊霞,郭嘉亮,等. 一种混沌伪随机序列均匀化普适算法的改进[J]. 电子学报,2015,43(4): 753-759. doi: 10.3969/j.issn.0372-2112.2015.04.018LI Peiyue, SHI Junxia, GUO Jialiang, et al. Improvement of a universal algorithm for uniformization of chaotic pseudo-random sequences[J]. Acta Electronica Sinica, 2015, 43(4): 753-759. doi: 10.3969/j.issn.0372-2112.2015.04.018 [21] WANG Xiaomin, ZHANG Jiashu. An image scrambling encryption using chaos-controlled Poker shuffle operation[C]//2008 International Symposium on Biometrics and Security Technologies. Isalambad: IEEE, 2008: 1-6. 期刊类型引用(4)
1. 张爽，李震. 基于耦合映像格子的明文关联图像加密方案. 聊城大学学报(自然科学版). 2025(01): 14-22 . 
百度学术2. 张爽，李震. 基于双超混沌系统的分块图像加密方案. 智能计算机与应用. 2024(04): 96-101 . 百度学术3. 白牡丹，赵莉，李珊珊. 基于压缩感知和超混沌系统的多图像加密算法. 计算机系统应用. 2023(02): 295-302 . 百度学术4. 王永，龚建，王明月. 基于三维分段Sine映射的彩色图像加密. 北京理工大学学报. 2022(04): 415-423 . 百度学术其他类型引用(6)
-
下载:
下载:
百度学术
点击查看大图
计量
- 文章访问数: 475
- HTML全文浏览量: 210
- PDF下载量: 28
- 被引次数: 10