Statistical Damage Model for Whole Deformation and Failure Process of Rock Considering Initial Void Closure
-
摘要:
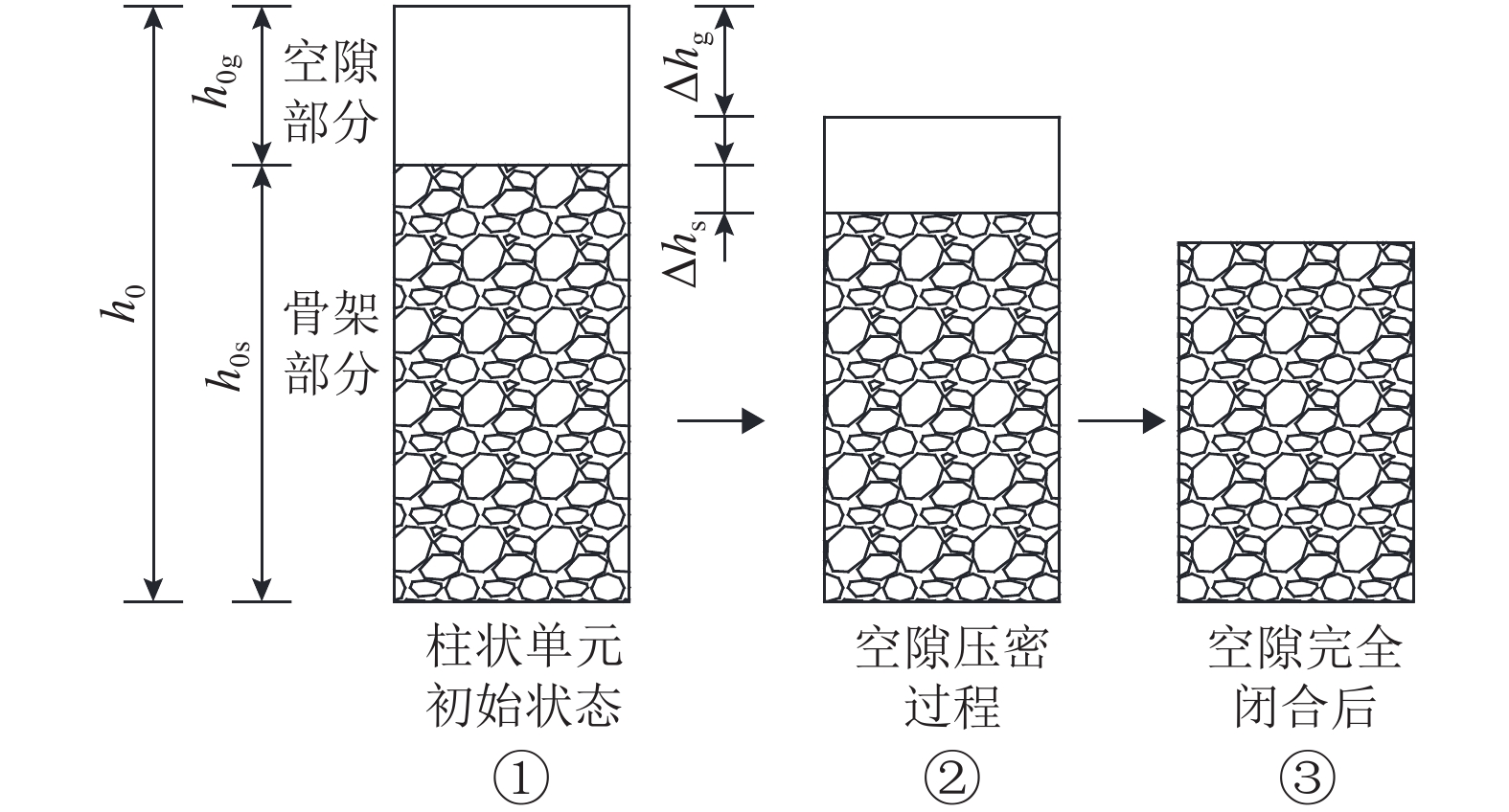
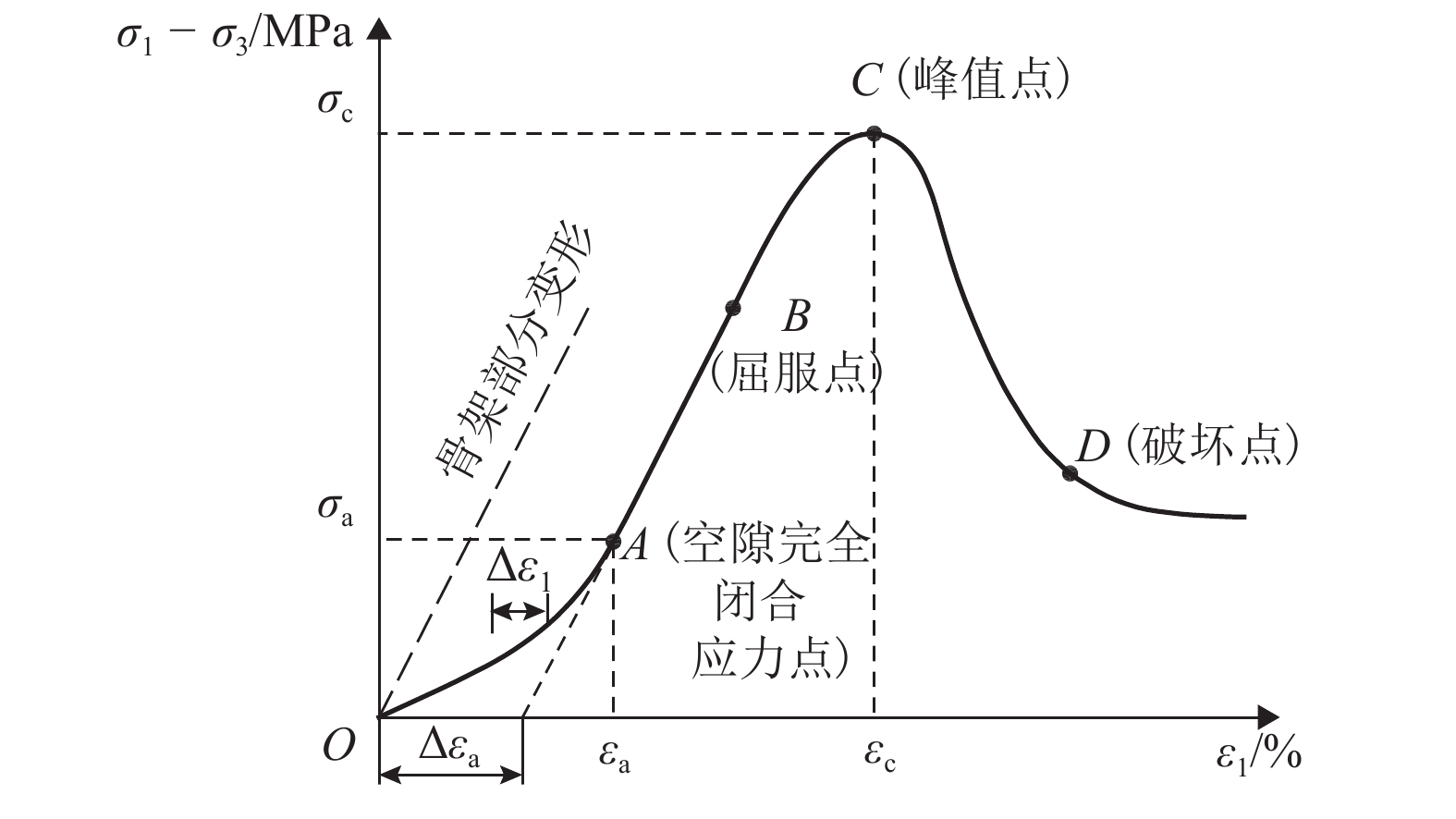
为了建立能够准确模拟岩石变形全过程的本构模型，深入分析了现有统计损伤模型难以描述岩石初始非线性变形阶段的局限性. 综合考虑岩石的变形机理，将岩石抽象为由空隙和骨架组成的材料，分析了岩石变形及空隙与骨架两部分变形之间的关系，提出了空隙应变比
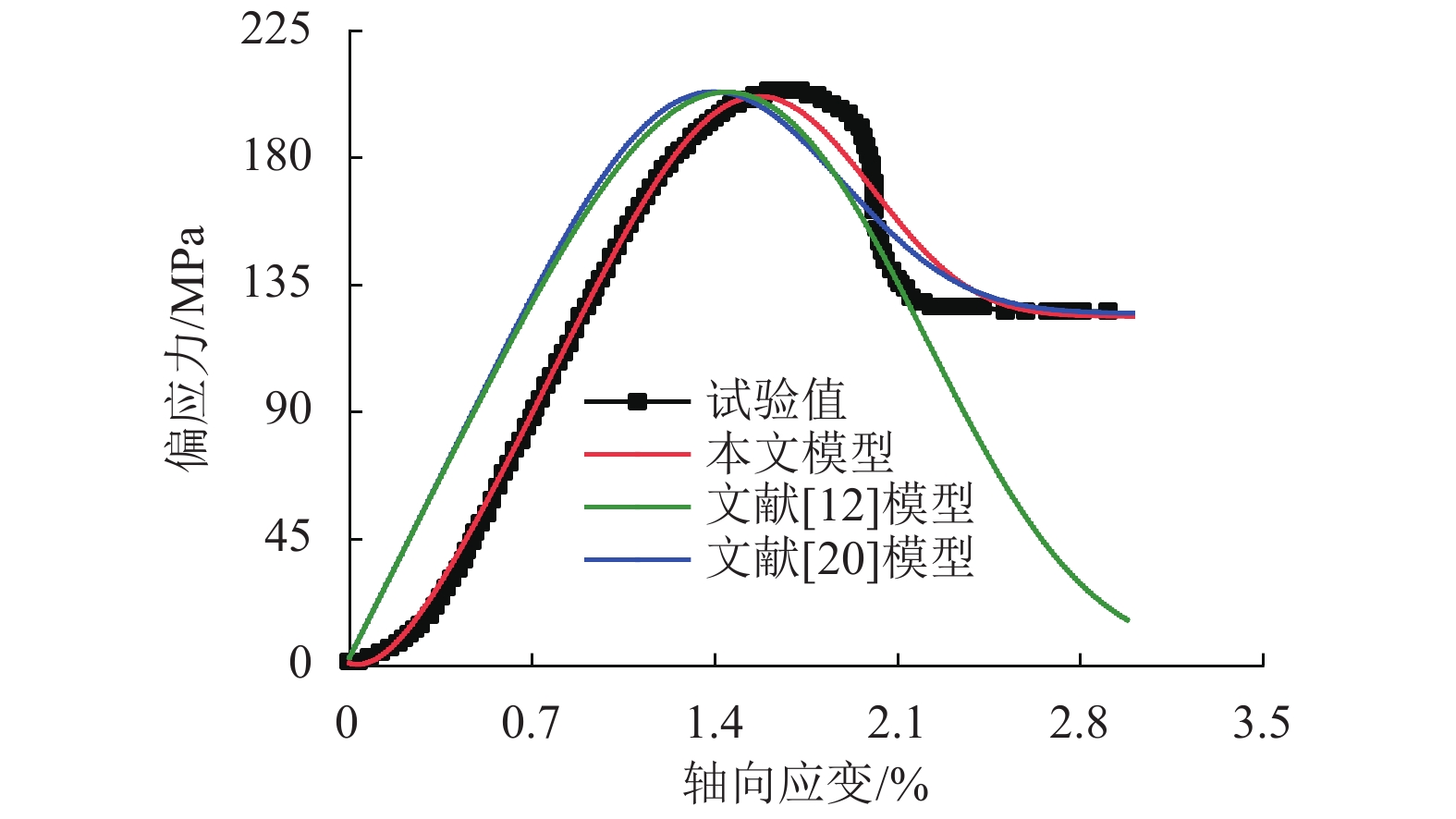
K 的概念；利用岩石三轴试验结果，提出了岩石骨架和空隙两部分应变的计算方法，推导了K 的演化方程；通过引入统计损伤理论，将岩石看作是由众多强度服从Weibull函数分布的微元组成，最终建立了能够反映岩石变形全过程的本构模型，并给出了模型相关参数的确定方法. 现有模型结果和试验结果比较分析表明：本文模型能够较好地模拟荷载作用下岩石变形破坏全过程的5个阶段，相关系数均在0.92以上，很好地解释了围压越大初始空隙压密阶段越短以及弹性模量、峰值强度和峰值应变均越大的力学行为特性.Abstract:In order to establish a constitutive model that can accurately simulate the whole deformation and failure process of rock, a deep analysis is made of the limitations of the existing statistical damage models incapable of well describing the initial nonlinear phase of rock deformation. Considering the deformation mechanism of rock comprehensively, the intact rock is abstracted as a material composed of rock skeleton and voids. Based on the analysis of the deformation relationships among the whole rock, rock skeleton and voids, the concept of rock void strain ratio
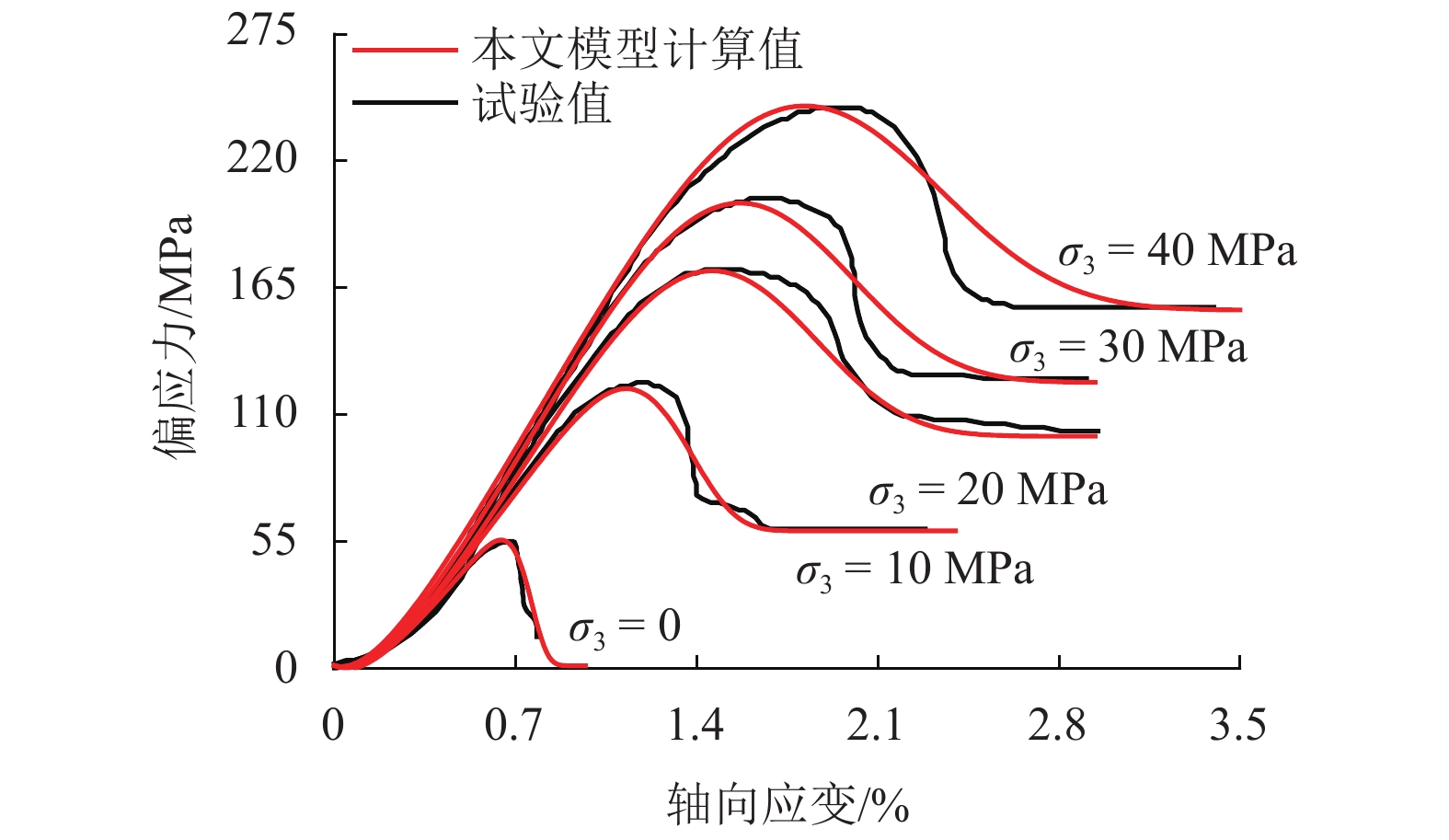
K is proposed; the calculation methods of rock skeleton strain and voids strain are presented using triaxial test results to derive the evolution equation ofK . By introducing the statistical damage theory, the rock is regarded as being composed of many micro elements whose strength obeys the distribution of Weibull function. Finally, a new constitutive model is established to describe the whole rock deformation process, and the determination methods of relevant model parameters are given. Compared with other model calculations and test results, this constitutive model can better simulate the five phases of the whole rock deformation and failure process under load, and the correlation coefficients are all larger than 0.92 for different confining test results. It also well explains the mechanical behavior characteristics that the larger the confining pressure, the shorter the initial void closure phase, and the larger the elastic modulus, peak strength and peak strain. -
图 8 不同m值对应的损伤变量D随ε/ε0的变化
Figure 8. Variation of damage variables D with ε/ε0 for different m
图 9 Weibull分布参数ε0与εc的关系
Figure 9. Relationship between peak strain εc and the parameter ε0 of Weibull distribution
图 10 本文模型计算结果与试验曲线之间的比较
Figure 10. Comparison between the proposed constitutive model calculation values and experimental curves
图 11 不同模型计算结果与试验值的比较
Figure 11. Different model calculations versus experimental curve
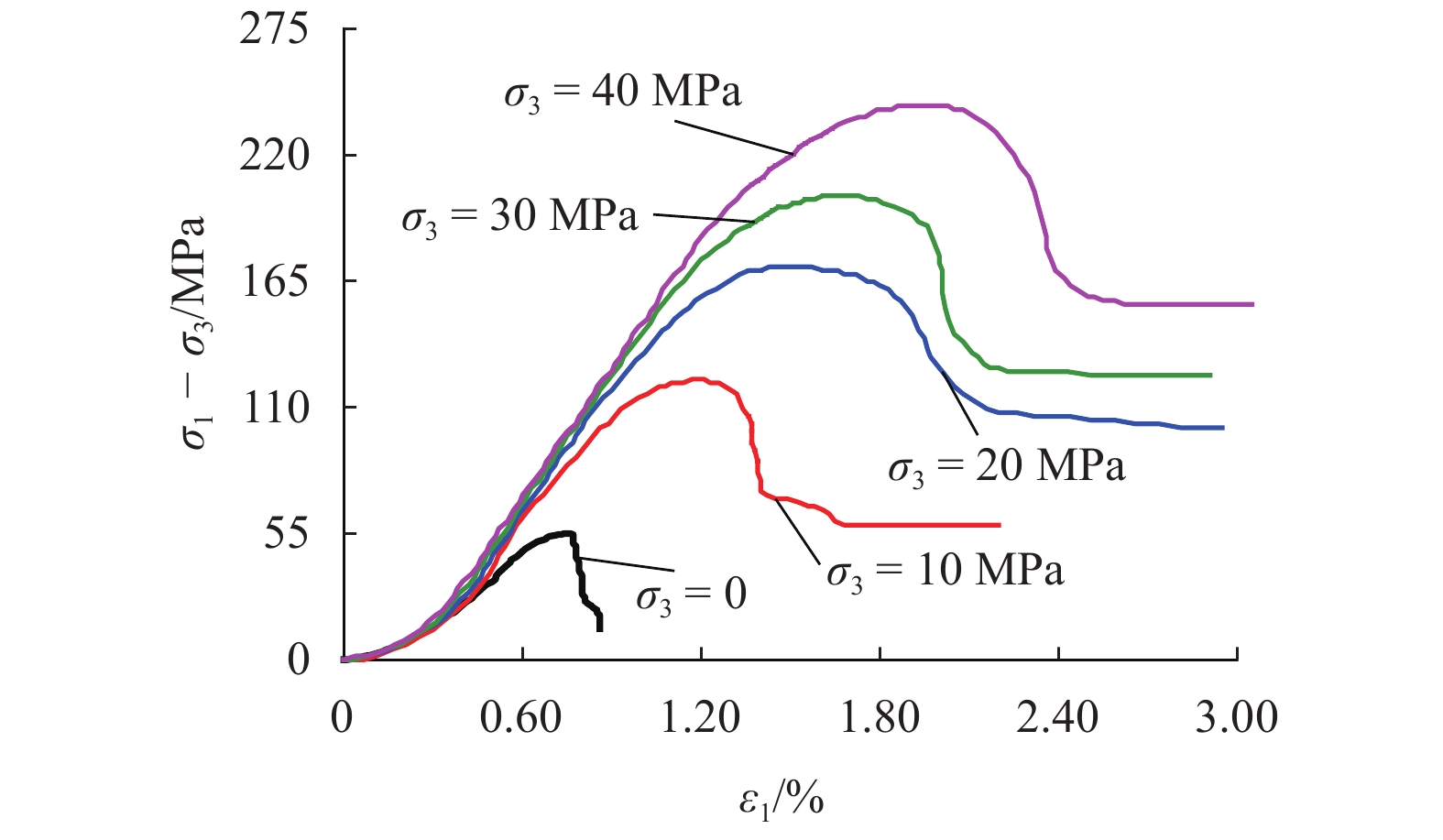
表 1 岩石三轴试验参数
Table 1. Triaxial test parameters for rocks
σ3
/MPaE
/MPaεa
/%σc
/MPaεc
/%R
/MPa0 13.72 0.487 54.92 0.697 0 10 14.68 0.443 122.38 1.235 57.7 20 16.17 0.425 171.2 1.57 101.5 30 18.12 0.409 201.17 1.762 123.8 40 19.28 0.374 243.36 1.993 154.9 
下载: 导出CSV
表 2 本文岩石统计损伤模型参数
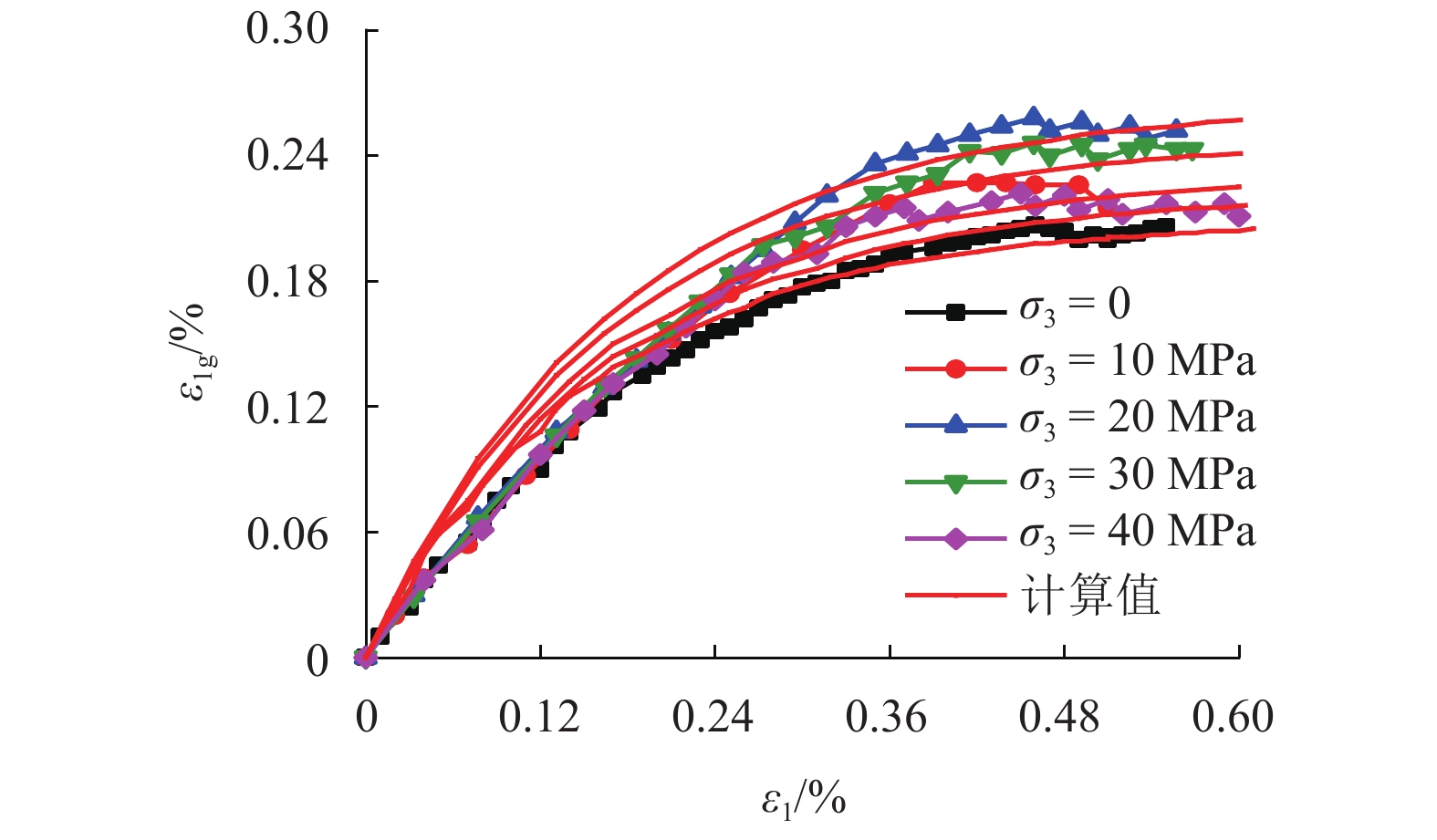
Table 2. Parameters of statistical damage model for rocks
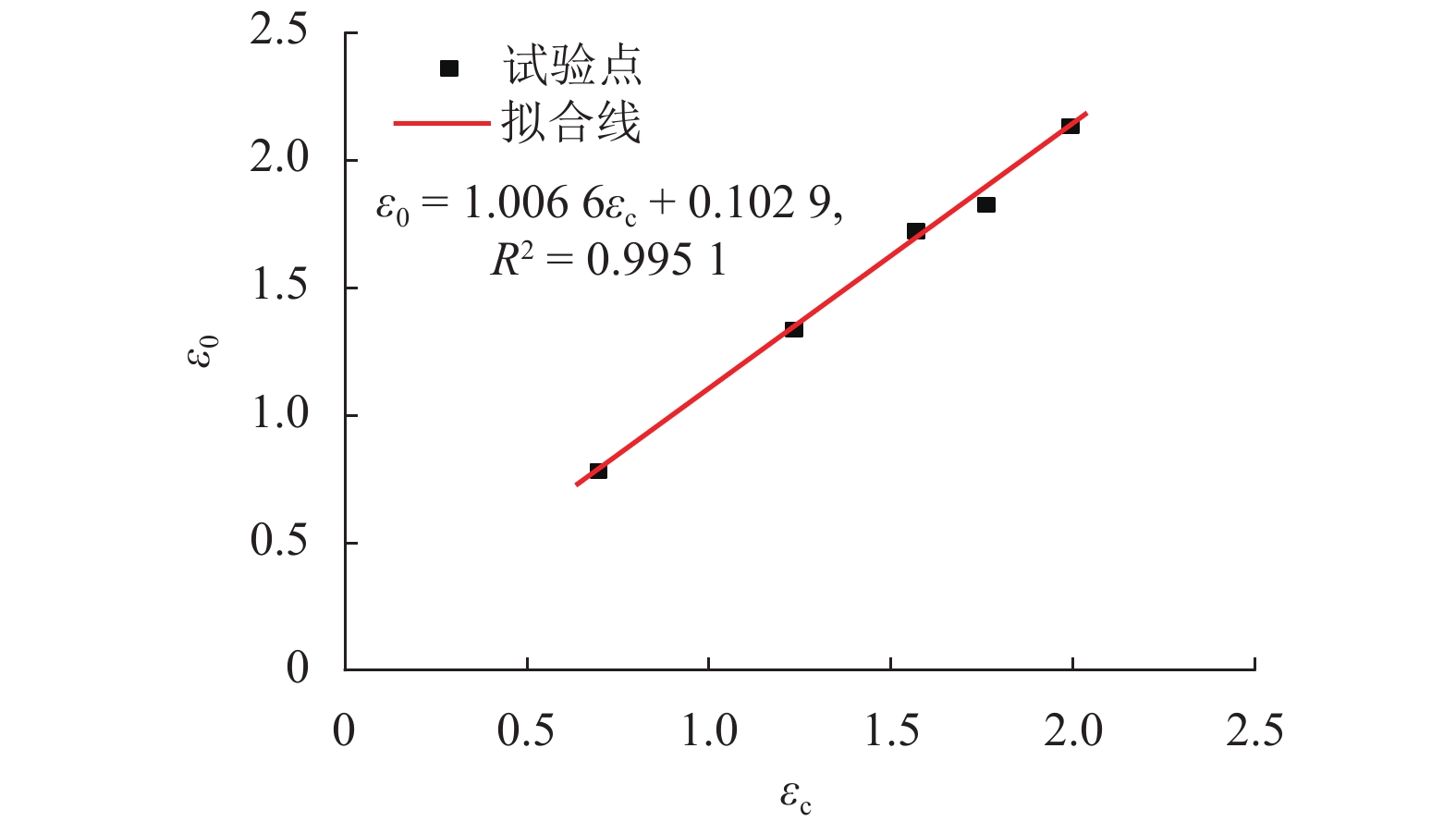
σ3 /MPa a1 a2 ε0/% m ε0－εc/% 0 0.203 6.301 0.782 14.258 0.095 10 0.226 5.965 1.337 7.576 0.102 20 0.252 5.694 1.714 4.735 0.144 30 0.249 5.379 1.827 4.581 0.065 40 0.217 5.283 2.134 4.269 0.141
下载: 导出CSV
-
[1] YANG S Q. Experimental study on deformation,peak strength and crack damage behavior of hollow sandstone under conventional triaxial compression[J]. Engineering Geology, 2016, 213: 11-24. doi: 10.1016/j.enggeo.2016.08.012 [2] 张春会,赵全胜,王来贵,等. 三轴压缩岩石应变软化及渗透率演化的试验和数值模拟[J]. 煤炭学报,2015,40(8): 1774-1782.ZHANG Chunhui, ZHAO Quansheng, WANG Laigui, et al. Test and numerical modeling on strain softening behavior and permeability evolution of rock under triaxial compression[J]. Journal of China Coal Society, 2015, 40(8): 1774-1782. [3] 衡帅,杨春和,张保平,等. 页岩各向异性特征的试验研究[J]. 岩土力学,2015,36(3): 609-616.HENG Shuai, YANG Chunhe, ZHANG Baoping, et al. Experimental research on anisotropic properties of shale[J]. Rock and Soil Mechanics, 2015, 36(3): 609-616. [4] 卢允德,葛修润,蒋宇,等. 大理岩常规三轴压缩全过程试验和本构方程的研究[J]. 岩石力学与工程学报,2004,23(15): 2489-2493. doi: 10.3321/j.issn:1000-6915.2004.15.001LU Yunde, GE Xiurun, JIANG Yu, et al. Study on conventional triaxial compression test of complete process for marble and its constitutive equation[J]. Chinese Journal of Rock Mechanics and Engineering, 2004, 23(15): 2489-2493. doi: 10.3321/j.issn:1000-6915.2004.15.001 [5] KRAJCINOVIC D, SILVA M A D. Statistical aspects of the continuous damage theory[J]. International Journal of Solid and Structure, 1982, 18(7): 551-562. doi: 10.1016/0020-7683(82)90039-7 [6] CHEN S, QIAO C S, YE Q, et al. Comparative study on three-dimensional statistical damage constitutive modified model of rock based on power function and Weibull distribution[J]. Environmental Earth Science, 2018, 77(3): 108-116. doi: 10.1007/s12665-018-7297-6 [7] 张慧梅,雷利娜,杨更社. 等围压条件下岩石本构模型及损伤特性[J]. 中国矿业大学学报,2015,44(1): 59-63.ZHANG Huimei, LEI Lina, YANG Gengshe. Characteristic and representation model of rock damage process under constant confining stress[J]. Journal of China University of Mining and Technology, 2015, 44(1): 59-63. [8] 张明,王菲,杨强. 基于三轴压缩试验的岩石统计损伤本构模型[J]. 岩土工程学报,2013,35(11): 1965-1971.ZHANG Ming, WANG Fei, YANG Qiang. Statistical damage constitutive model for rocks based on triaxial compression tests[J]. Chinese Journal of Geotechnical Engineering, 2013, 35(11): 1965-1971. [9] WEN T, LIU Y R, YANG C G, et al. A rock damage constitutive model and damage energy dissipation rate analysis for characterising the crack closure effect[J]. Geomechanics and Geoengineering, 2018, 13(1): 54-63. doi: 10.1080/17486025.2017.1330969 [10] ZHOU S W, XIA C C, ZHAO H B, et al. Statistical damage constitutive model for rocks subjected to cyclic stress and cyclic temperature[J]. Acta Geophy, 2017, 65(5): 893-906. doi: 10.1007/s11600-017-0073-2 [11] LEMAITRE. How to use damage mechanics[J]. Nuclear Engineering and Dwsign, 1984, 80(2): 233-245. doi: 10.1016/0029-5493(84)90169-9 [12] 曹文贵,张升,赵明华. 软化与硬化特性转化的岩石损伤统计本构模型之研究[J]. 工程力学,2006,23(11): 110-115. doi: 10.3969/j.issn.1000-4750.2006.11.018CAO Wengui, ZHANG Sheng, ZHAO Minghua. Study on a statistical damage constitutive model with conversion between softening and hardening properties of rock[J]. Engineering Mechanics, 2006, 23(11): 110-115. doi: 10.3969/j.issn.1000-4750.2006.11.018 [13] 李海潮,张升. 基于修正Lemaitre应变等价性假设的岩石损伤模型[J]. 岩土力学,2017,38(5): 1321-1326.LI Haichao, ZHANG Sheng. A constitutive damage model of rock based on the assumption of modified Lemaitre strain equivalence hypothesis[J]. Rock and Soil Mechanics, 2017, 38(5): 1321-1326. [14] 刘冬桥,王焯,张晓云. 岩石应变软化变形特性及损伤本构模型研究[J]. 岩土力学,2017,38(10): 2901-2908.LIU Dongqiao, WANG Zhou, ZHANG Xiaoyun. Characteristics of strain softening of rocks and its damage constitutive model[J]. Rock and Soil Mechanics, 2017, 38(10): 2901-2908. [15] LI Y W, JIA D, RUI Z H, et al. Evaluation method of rock brittleness based on statistical constitutive relations for rock damage[J]. Journal of Petroleum Science and Engineering, 2017, 153: 123-132. doi: 10.1016/j.petrol.2017.03.041 [16] 曹文贵,张超,贺敏,等. 考虑孔隙压密阶段特征的岩石应变软化统计损伤模拟方法[J]. 岩土工程学报,2016,38(10): 1754-1761. doi: 10.11779/CJGE201610002CAO Wengui, ZHANG Chao, HE Min, et al. Statistical damage simulation method of strain softening deformation process for rock considering characteristics of void compaction stage[J]. Chinese Journal of Geotechnical Engineering, 2016, 38(10): 1754-1761. doi: 10.11779/CJGE201610002 [17] XU P, YANG S Q. A fracture damage constitutive model for fissured rock mass and its experimental verification[J]. Arabian Journal of Geosciences, 2017, 10(7): 2947-2954. [18] MENENDEZ B, ZHU W, WONG T F. Micromechanics of brittle faulting and cataclastic flow in Berea sandstone[J]. Journal of Structural Geology, 1996, 18(1): 1-16. doi: 10.1016/0191-8141(95)00076-P [19] HAJIAOBDOLMAJID V, KAISER P K, MARTIN C D. Modelling brittle failure of rock[J]. International Journal of Rock Mechanics and Mining Sciences, 2002, 39(6): 731-741. [20] ZHAO H, SHI C J, ZHAO M H, et al. Statistical damage constitutive model for rocks considering residual strength[J]. International Journal of Geomechanics, 2017, 17(1): 04016033.1-04016033.9. -
下载:
点击查看大图
计量
- 文章访问数: 298
- HTML全文浏览量: 174
- PDF下载量: 38
- 被引次数: 0