Analysis of Astrometry Accuracy Considering Effects of Bulletin A
-
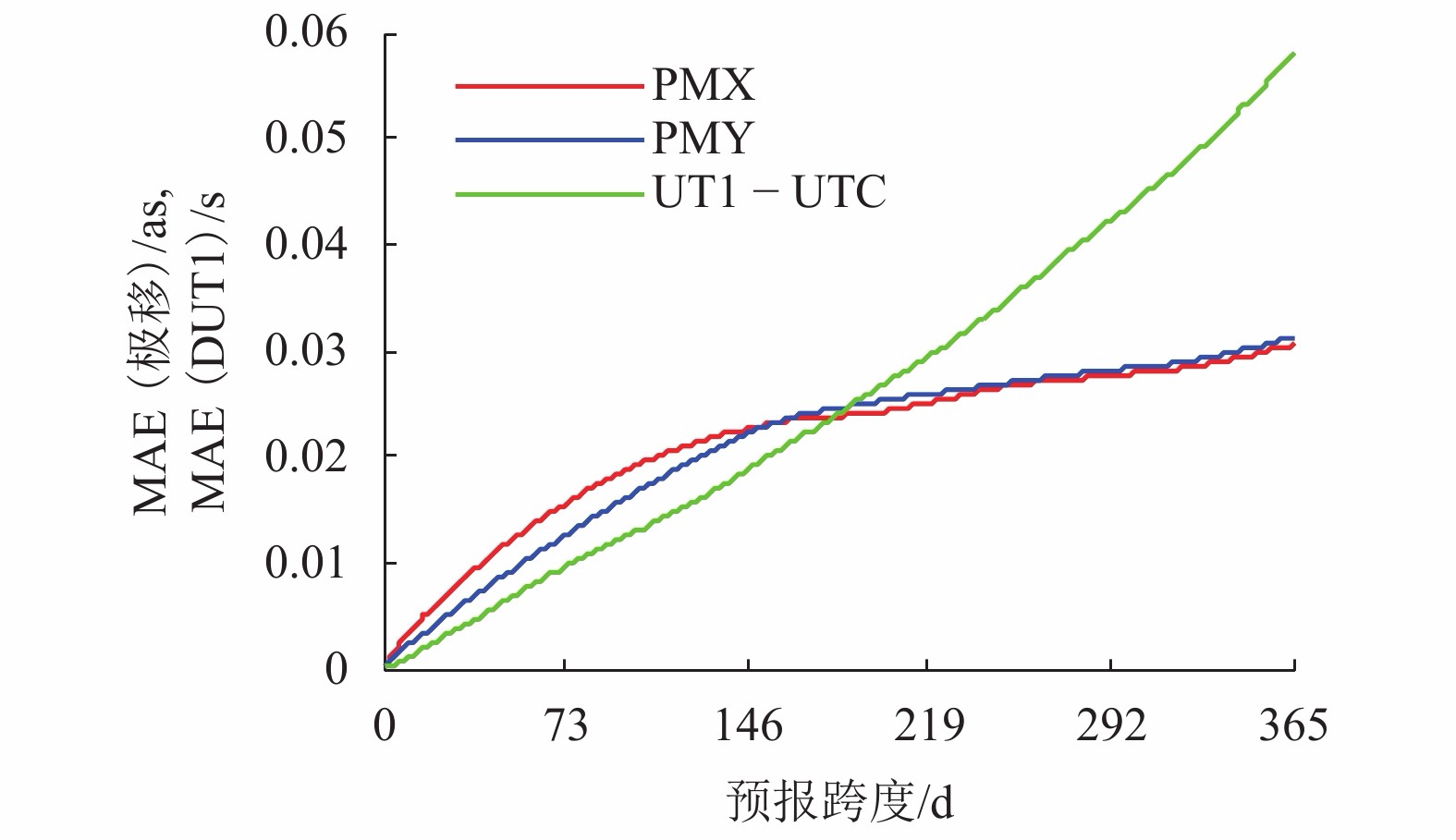
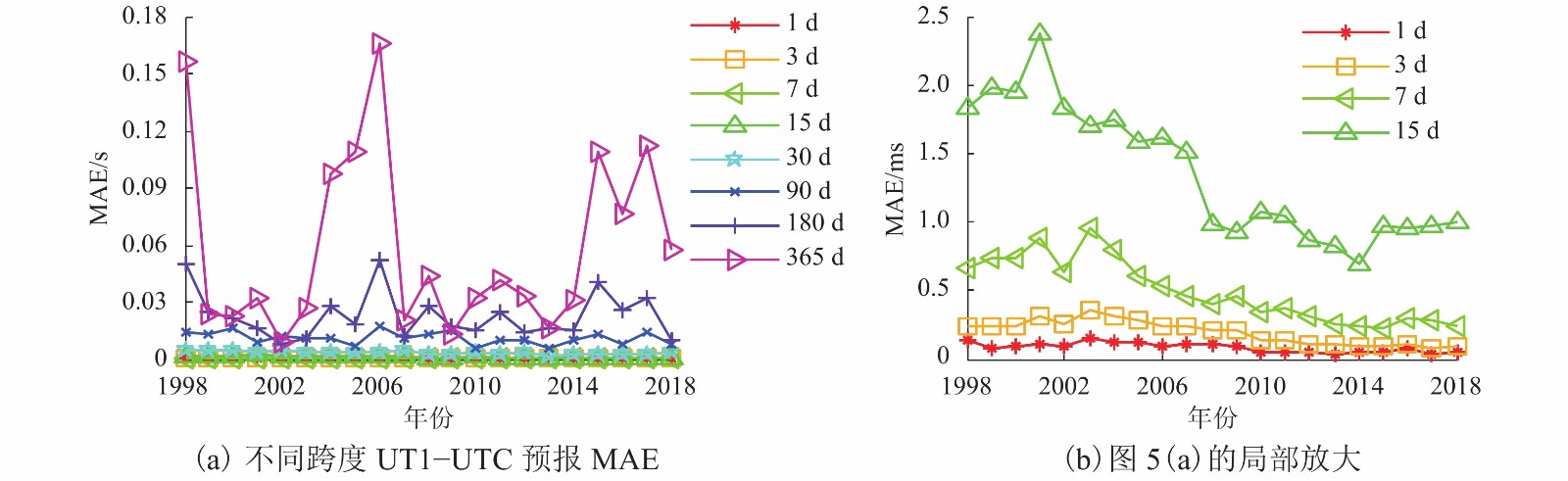
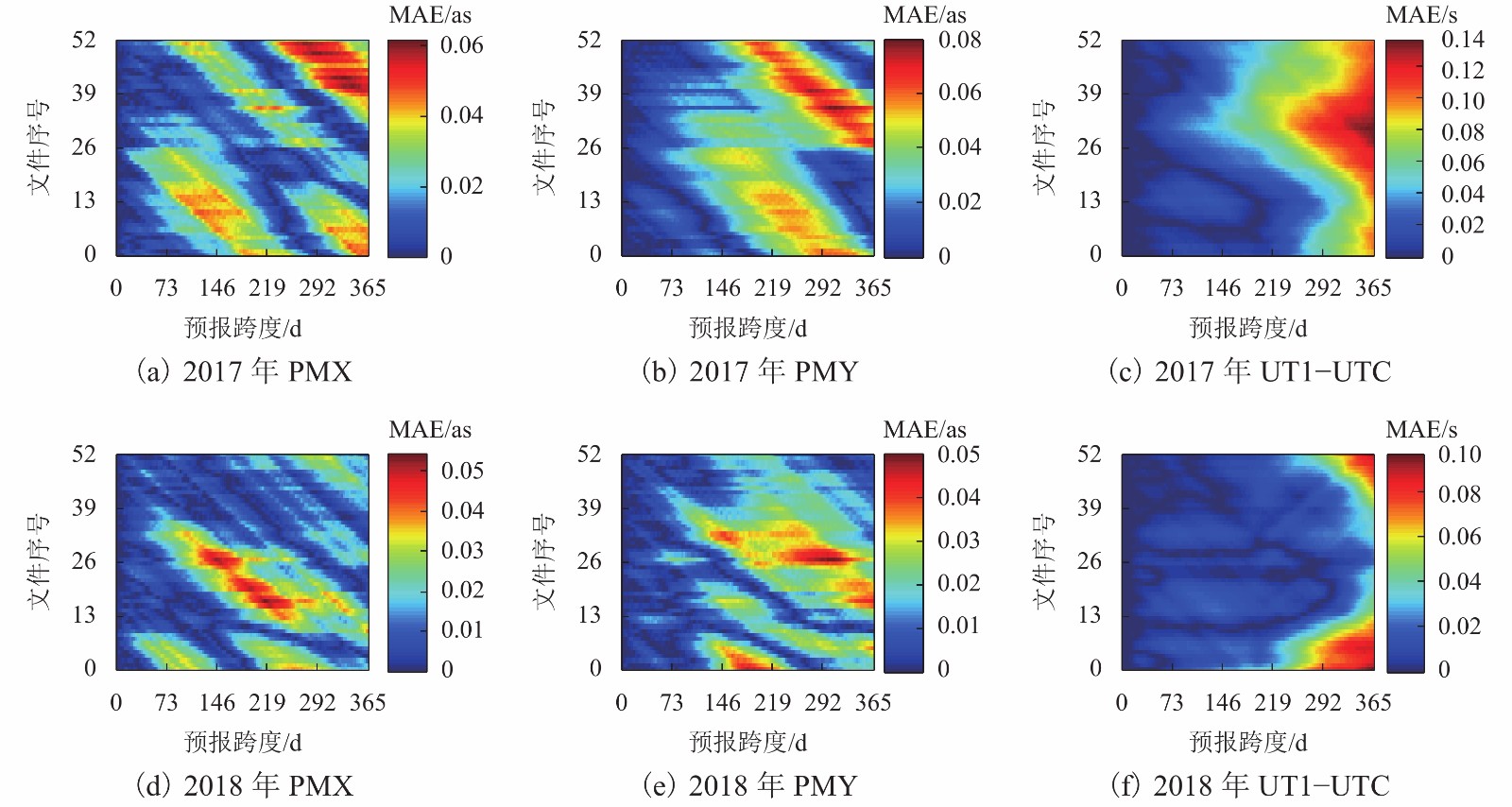
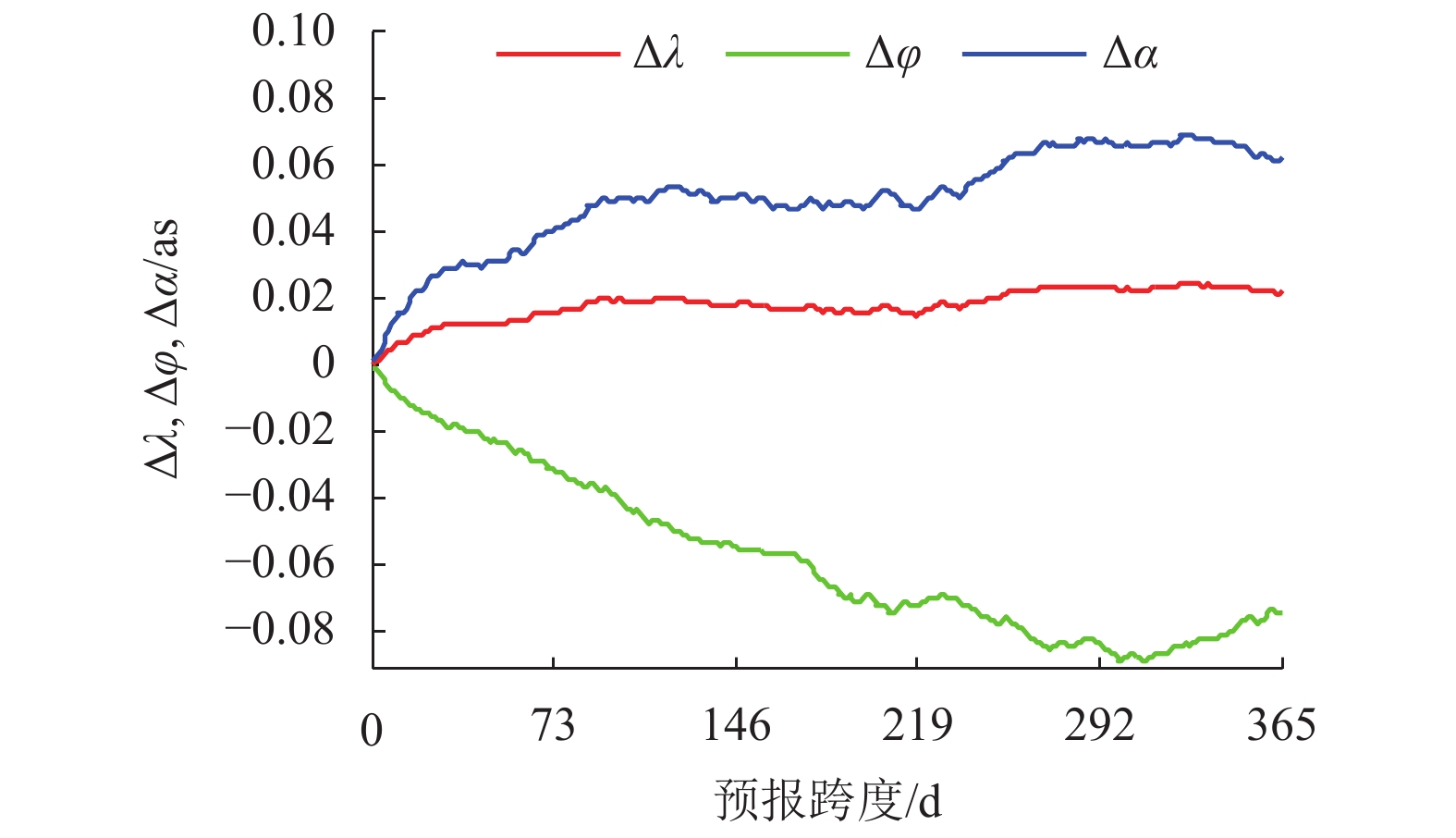
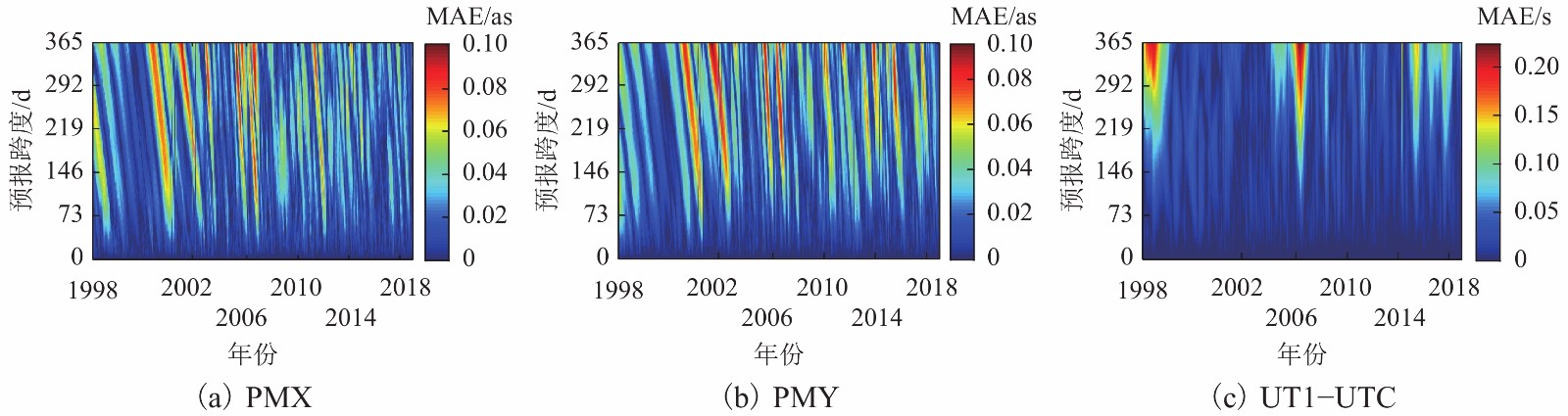
摘要: 地球定向参数是解算天文测量数据的重要参数，其精度直接影响最终的定位定向结果. 目前，没有一种技术手段可以实时获取高精度的EOP （earth orientation parameters）观测值，主要使用国际地球自转和参考系服务（International Earth Rotation and Reference Systems Service，IERS）发布的Bulletin A （简称A公报）预报值进行解算，但其对天文测量影响尚缺少系统的研究. 为此，研究了1998年—2018年每年1～365 d预报误差的长期变化情况及不同时间跨度的预报误差变化情况，并以某站数字天顶仪观测数据为例分析了预报误差对天文测量的影响. 分析结果表明：1年跨度的极移预报值对天文经度的影响小于0.03 as，纬度的影响小于0.09 as，天文方位角小于0.07 as，该指标远高于一等天文测量的精度要求；UT1−UTC （universal time1−universal time coordinated）的预报精度是限制A公报精度主要因素，其40 d以上的预报值无法满足一等天文测量的最低要求，预报精度仍有待于进一步提升.Abstract: Earth orientation parameters (EOP) is of very importance to astronomical data calculation and its accuracy affects the final positioning and orientation. At present, there is no technical means to obtain high-precision EOP observation value in real time, which mainly relies on the Bulletin A forecast issued by the International Earth Rotation and Reference Systems Service (IERS). However, there is still lack of systematic research on how it affects astronomical measurement. To this end, the long-term variation of forecast error and the variation of forecast error for different time spans from 1 to 365 days are studied for a period of 1998—2018. Taking the observation data of a digital zenith telescope as an example, the influence of forecast error on astronomical measurement is analyzed. It shows that the influence of polar motion forecast for 365 days on astronomical longitude is less than 0.03 as, on latitude less than 0.09 as, and on astronomical azimuth less than 0.07 as, showing that it outperforms the precision requirement of first order astrometry. UT1−UTC (universal time1−universal time coordinated) forecast accuracy is the main factor limiting A bulletin accuracy. The forecast accuracy of the UT1−UTC is the main factor limiting the accuracy of the Bulletin A, and the error of its prediction over 40 days can not meet the minimum requirements of the first order astrometry, and the prediction accuracy of the UT1−UTC still needs to be further improved.
-
Key words:
- earth rotation parameters /
- geodetic astrometry /
- forecast error /
- polar motion /
- UT1−UTC
-
图 2 1998年—2018年A公报MAE随预报跨度变化
Figure 2. MAE variation from Bulletin A with time spans from 1998 to 2018
图 3 1998年—2018年不同跨度PMX预报 MAE随时间变化
Figure 3. MAE variation of PMX with different time spans form 1998 to 2018
图 4 1998年—2018年不同跨度PMY预报MAE随时间变化
Figure 4. MAE variation of PMY with different time spans form 1998 to 2018
图 5 1998年—2018年不同跨度UT1−UTC预报MAE随时间变化
Figure 5. MAE variation of UT1−UTC with different time spans form 1998 to 2018
图 6 2017年、2018年A公报预报误差分布
Figure 6. Distribution of forecast errors in Bulletin A in 2017 and 2018
图 7 2017年极移预报误差对天文定位定向的影响
Figure 7. Effect of polar motion forecast error on astronomical positioning and orientation in 2017
图 8 2018年极移预报误差对天文定位定向的影响
Figure 8. Effect of polar motion forecast error on astronomical positioning and orientation in 2018
图 9 2017年、2018年UT1−UTC预报误差对经度影响
Figure 9. Effect of UT1−UTC forecast error on longitude in 2017 and 2018
表 1 2017年、2018年A公报预报统计
Table 1. Statistics of Bulletin A in 2017 and 2018
年份 参数 误差 时间跨度/d 1 3 7 15 30 90 180 365 2018 年 PMX/mas 最大绝对误差 1.32 3.70 7.50 12.90 21.10 33.40 46.70 40.70 MAE 0.26 0.75 1.97 3.86 6.69 13.50 17.20 14.30 中误差 0.35 0.94 2.36 4.51 7.38 12.50 19.50 16.50 PMY/mas 最大绝对误差 0.55 2.26 4.53 8.05 13.36 21.50 44.40 42.00 MAE 0.17 0.61 1.45 2.60 4.13 7.40 20.10 17.90 中误差 0.22 0.81 1.81 3.18 5.04 9.30 18.60 19.10 UT1−UTC/ms 最大绝对误差 0.15 0.30 0.61 2.89 9.03 17.80 32.97 97.14 MAE 0.05 0.09 0.24 1.00 3.32 8.20 9.95 57.20 中误差 0.06 0.11 0.29 1.25 4.03 9.80 11.10 22.60 2017年 PMX/mas 最大绝对误差 1.04 2.83 9.39 16.78 26.40 43.30 43.20 55.60 MAE 0.28 0.91 2.19 4.50 7.61 20.10 22.50 29.20 中误差 0.37 1.10 2.78 5.25 7.59 12.80 12.20 25.70 PMY/mas 最大绝对误差 0.61 1.94 4.70 8.13 13.70 28.97 56.90 65.10 MAE 0.19 0.67 1.39 2.58 4.88 14.70 35.20 23.60 中误差 0.24 0.83 1.86 3.25 5.77 13.80 21.10 27.50 UT1−UTC/ms 最大绝对误差 0.16 0.26 0.87 3.39 8.20 34.50 65.00 136.50 MAE 0.04 0.08 0.29 0.98 2.92 13.70 31.80 111.90 中误差 0.05 0.11 0.27 1.10 3.65 14.70 24.00 13.30 
下载: 导出CSV
表 2 大地天文测量的精度指标
Table 2. Precision indexes in geodetic astrometry
坐标 等 级 一等 二等 三等 四等 经度/as 0.30 0.60 1.20 6.00 纬度/as 0.30 0.50 1.00 5.00 方位角/as 0.50 1.00 5.00 10.00
下载: 导出CSV
-
王若璞, 张超, 李崇辉. 大地天文测量原理与方法[M].北京: 测绘出版社, 2018: 2-3 MICHAEL S, RALF L. 时空参考系[M]. 王若璞, 赵东明, 译. 北京: 科学出版社, 2015: 6-7. 牛国华, 郑晓龙, 李雪瑞, 等.大地天文测量[M]. 北京: 国防工业出版社, 2016: 7-8. 郑伟, 陈小前, 杨希祥. 天文学基础[M]. 北京: 国防工业出版社.2015: 4-8. KALARUS M, SCHUH H, KOSEK W, et al. Achievements of the Earth orientation parameters prediction comparison campaign[J]. Journal of Geodesy, 2010, 84(10): 587-596. KOSEK W I, POPINSKI W, RZESZÓTKO A, et al. Causes of prediction errors of pole coordinates data[M]. [S.l.]: Wydawnictwo Centrum Badań Kosmicznych, Polska Akademia Nauk, 2009. KOSEK W. Future improvements in EOP prediction[C]//KENYON S, PACINO M, MARTI U. Geodesy for Planet Earth. Berlin: Springer, 2012(136): 513-520. 时春霖, 杨培章, 张超, 等. 基于Kittler最小误差分割算法的小视场星图分割[J]. 西南交通大学学报, 2021, 56(1): 168-175.SHI Chunlin, YANG Peizhang, ZHANG Chao, et al.Segmentation of small-field-of-viewstar images based on Kittler minimum error algorithm[J]. Journal of Southwest Jiaotong University, 2021, 56(1): 168-175. 时春霖,张超,袁晓波,等. 天文大地测量的发展现状和展望[J]. 测绘工程,2019,28(2): 37-44.SHI Chunlin, ZHANG Chao, YUAN Xiaobo. The present situation and prospect of astronomical geodetic measurement[J]. Engineering of Surveying and Mapping, 2019, 28(2): 37-44. 时春霖,张超,陈长远,等. 测量机器人小视场星图一维最大熵星点图像分割算法[J]. 测绘学报,2018,47(4): 26-34.SHI Chunlin, ZHANG Chao, CHEN Changyuan, et al. One dimensional maximum entropy star image segmentation algorithm for small field star image of measuring robot[J]. Acta Geodaetica et Cartographica Sinica, 2018, 47(4): 26-34. 李广宇. 天体测量与天体力学[M]. 北京: 科学出版社, 2015: 38-39. 张捍卫,许厚泽,王爱生. 天球参考系与地球参考系之间的坐标转换研究进展[J]. 测绘科学,2005,30(5): 105-109. doi: 10.3771/j.issn.1009-2307.2005.05.038ZHANG Hanwei, XU Houze, WANG Aisheng. The progress in transformation between the celestial and terrestria reference systems[J]. Science of Surveying and Mapping, 2005, 30(5): 105-109. doi: 10.3771/j.issn.1009-2307.2005.05.038 GAMBIS, D, LUZUM, B. Earth rotation monitoring,UT1 determination and prediction earth rotation monitoring,UT1 determinaiton and prediction[J]. Metrologia, 2011, 48(4): 165-170. doi: 10.1088/0026-1394/48/4/S06 宁津生, 刘经南, 陈俊勇, 等. 现代大地测量理论与技术[M]. 武汉: 武汉大学出版社, 2006: 170-171. LUZUM B J, RAY J R, CARTER M S, et al. Recent improvements to IERS Bulletin A combination and prediction[J]. GPS Solutions, 2001, 4(3): 34-40. doi: 10.1007/PL00012853 MCCARTHY D D, LUZUM B J. Prediction of earth orientation[J]. Bulletin Geodesique, 1991, 65(1): 18-21. doi: 10.1007/BF00806338 PETIT G, LUZUM B. IERS conventions (2010)[J]. IERS technical note 36[DB/OL]. [2020-01-20]. http://www.iers.org/IERS/EN/Publications/TechnicalNotes/tn36.html/. LAMBERT S, BIZOUARD C. Positioning the terrestrial ephemeris origin in the international terrestrial frame[J]. Astronomy & Astrophysics, 2002, 394: 317-321. CAPITAINE N, GOUINOT B, MCCARTHY D. Definition of the celestial ephemeris origin and of UT1 in the international Celestial reference frame[J]. Astronomy & Astrophysics, 2000, 355: 398-405. KOSEK W, KALARUS M, JOHNSON T J, et al. A comparison of LOD and UT1−UTC forecasts by different combined prediction techniques[J]. Artificial Satellites: a Journal of Planetary Geodesy, 2005, 40(2): 119-125. JOHNSON T J, LUZUM B J, RAY J R . Improved near-term Earth rotation predictions using atmospheric angular momentum analysis and forecasts[J]. Journal of Geodynamics, 2005, 39(3): 209-221. NIEDZIELSKI T, KOSEK W. Prediction of UT1–UTC,LOD and AAM χ3 by combination of least-squares and multivariate stochastic methods[J]. Journal of Geodesy, 2008, 82(2): 83-92. doi: 10.1007/s00190-007-0158-9 国家质量技术监督局. 大地天文测量规范: GB/T 17943—2000[S]. 北京: 中国标准出版社, 2000. -
下载:
点击查看大图
计量
- 文章访问数: 507
- HTML全文浏览量: 231
- PDF下载量: 13
- 被引次数: 0