Mechanical Properties of Tension-Torsion Coupling in Aluminum Conductor Steel Reinforced
-
摘要: 导线扭转参数属于导线基本力学性能之一,尤其是拉扭耦合效应会极大地影响覆冰输电线舞动分析的准确性. 为此,对典型7股钢芯铝绞线LGJ/JL/G1A-70/10进行了扭转试验,并结合有限元仿真软件ANSYS对相应构件进行建模与数值分析,与基于钢丝绳拉扭耦合理论的4种理论进行了对比. 数值分析结果与扭转试验结果吻合较好,且对比分析表明:拧绕系数的取值浮动较大,会导致不容忽视的误差;在正常运行应力状态下,导线拉伸会产生较大的扭转效应,导线的截面扭转也会产生轻微张力变化;导线的拉扭耦合和扭拉耦合系数不相等;基于钢丝绳的理论均未考虑子股导线的滑移变形及坐标更新,会在一定程度上高估轴向刚度以及拉扭耦合效应.Abstract: The torsion parameter of the conductor is one of its basic mechanical properties. Moreover, the tension-torsioncoupling effect of iced transmission lines will greatly affect the accuracy of galloping analysis. To analyze this effect, the torsional test was carried out for the typical 7-strand aluminum conductor steel reinforced (ACSR) LGJ/JL/G1A-70/10, and the modeling and numerical analysis of the corresponding components are carried out by using the finite element simulation software ANSYS, the results ofwhich is compared with those calculated by the 4 theories based on the tension-torsioncoupling theory of wire rope. The results of numerical analysis are in good agreement with those of the torsion test. Thetwist factor values fluctuate greatly, which will lead to significant errors. Under the normal stress state, the conductor tension will produce a large torsion effect, and the section torsion of the conductor will also lead to a slight tension change. The tension-torsion coupling coefficient is not equal to the torsion-tension one. The theories based on steel wire rope do not consider the slip deformation and coordinate update of sub strand conductors, which overestimates the axial stiffness and the coupling effect of tension and torsion to a certain extent.
-
表 1 钢芯铝绞线LGJ/JL/G1A-70/10的物理参数
Table 1. Physical parameters of wires
参数 值 根 × 直径/mm 钢 1 × 3.80 铝 6 × 3.80 截面积/mm2 钢/铝 11.3/68.0 总截面 79.3 铝、钢截面比 6.02 直径/mm 11.4 单位质量/(t•km−1) 0.275 计算拉断力/kN 23.36 综合弹性模量/MPa 74300 节距/mm 13 线膨胀系数/(℃−1) 18.8 × 10−6 表 2 试件剪切模量
Table 2. Shear modulus of test specimens
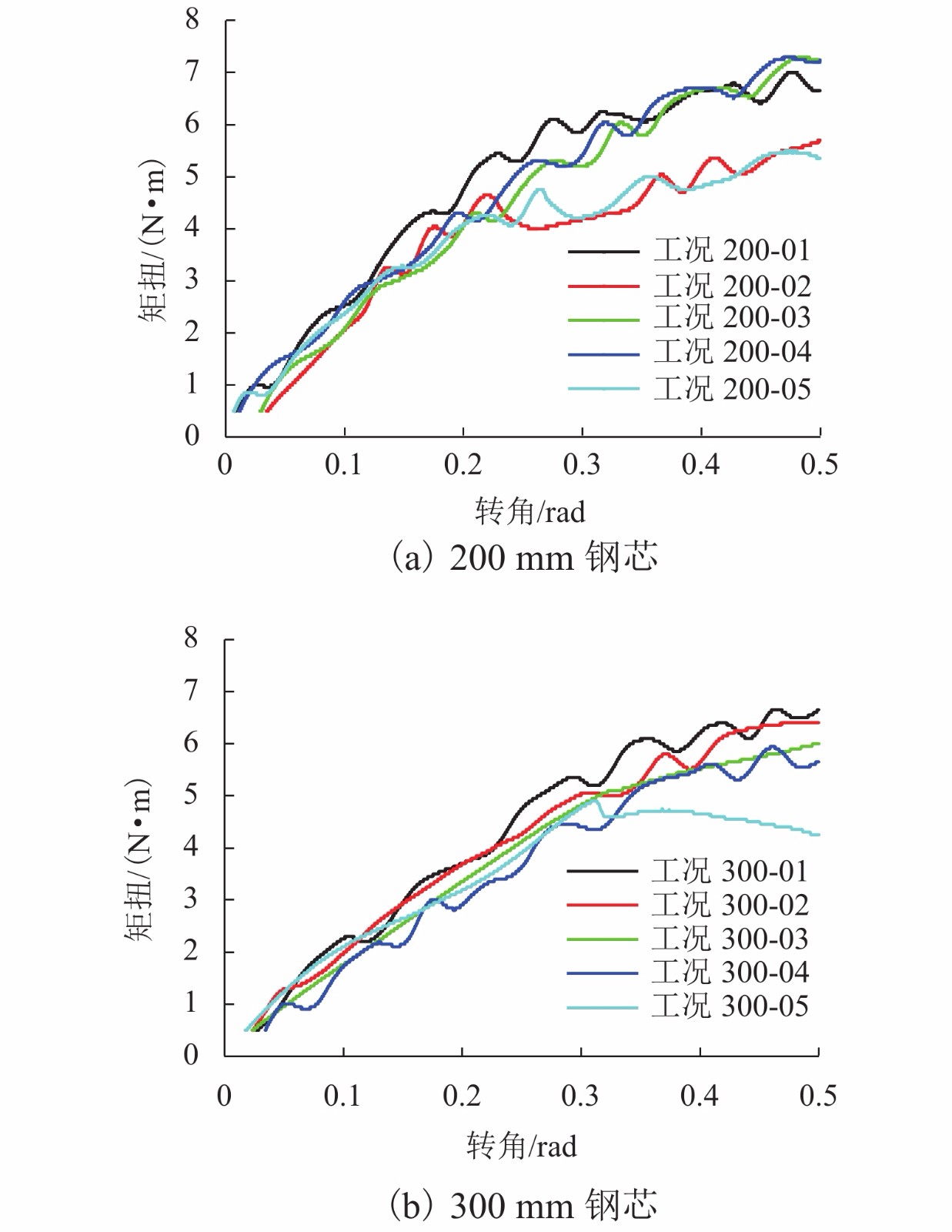
工况 ¯J/mm4 ¯G/GPa 200-01 1125.893 4.378 200-02 1125.893 4.745 200-03 1125.893 3.882 200-04 1125.893 3.761 200-05 1125.893 4.684 300-01 1125.893 4.659 300-02 1125.893 4.565 300-03 1125.893 4.268 300-04 1125.893 4.058 300-05 1125.893 3.734 平均值 1125.893 4.405 表 3 纯扭下的轴力与扭矩
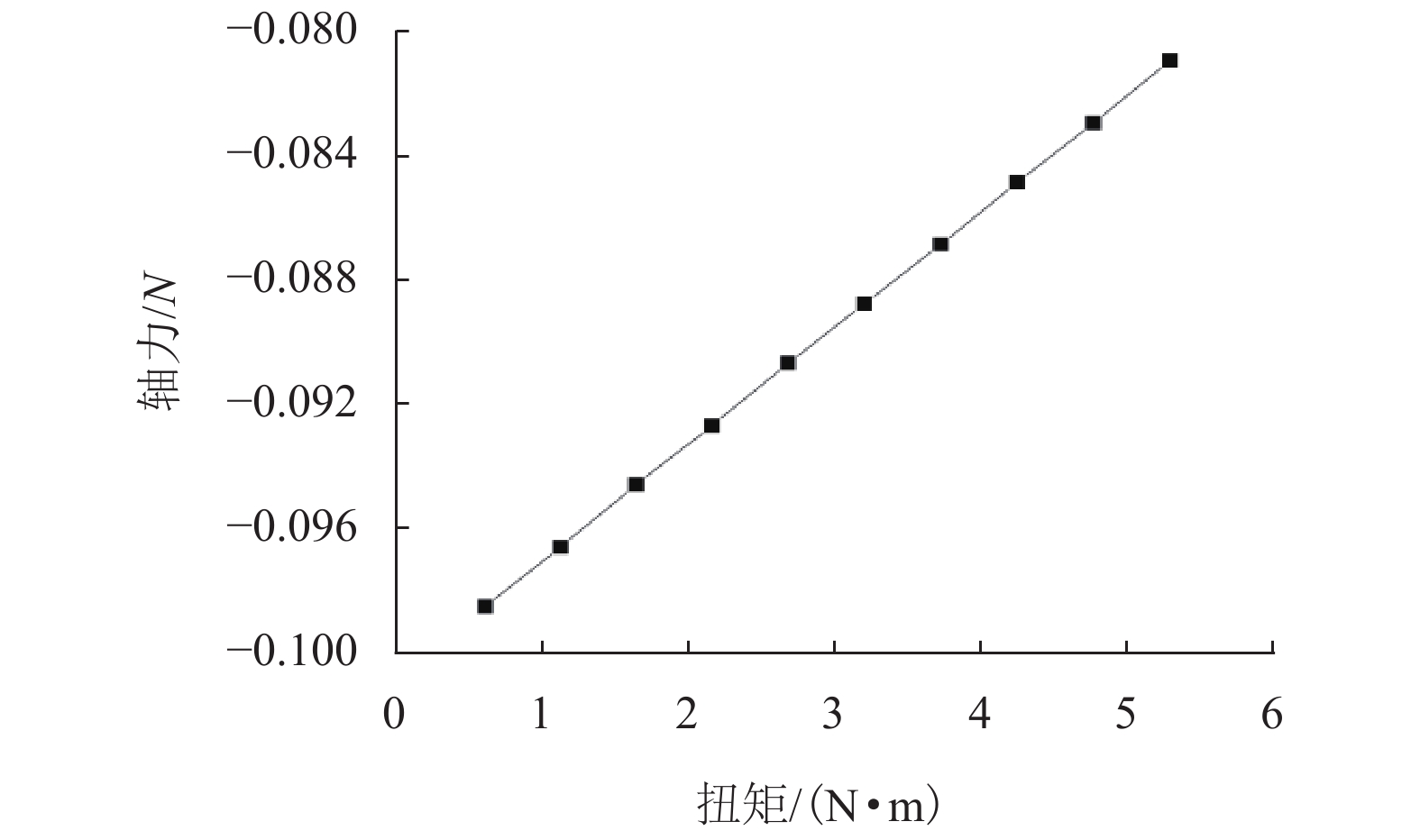
Table 3. Axis force and torque under pure torsion
转角/
radS-轴
力/NS-扭矩/
(N•m)L-轴
力/NL-扭矩/
(N•m)平均扭矩/
(N•m)0.02 0.099 0.628 0.099 0.630 0.607 0.04 0.097 1.138 0.097 1.141 1.099 0.06 0.096 1.648 0.095 1.654 1.593 0.08 0.094 2.160 0.093 2.167 2.088 0.10 0.092 2.672 0.091 2.682 2.584 0.12 0.090 3.185 0.089 3.198 3.081 0.14 0.088 3.699 0.087 3.715 3.579 0.16 0.086 4.213 0.085 4.232 4.078 0.18 0.084 4.728 0.083 4.751 4.578 0.20 0.082 5.243 0.081 5.270 5.078 表 4 刚度方程对比
Table 4. Comparison of stiffness equation
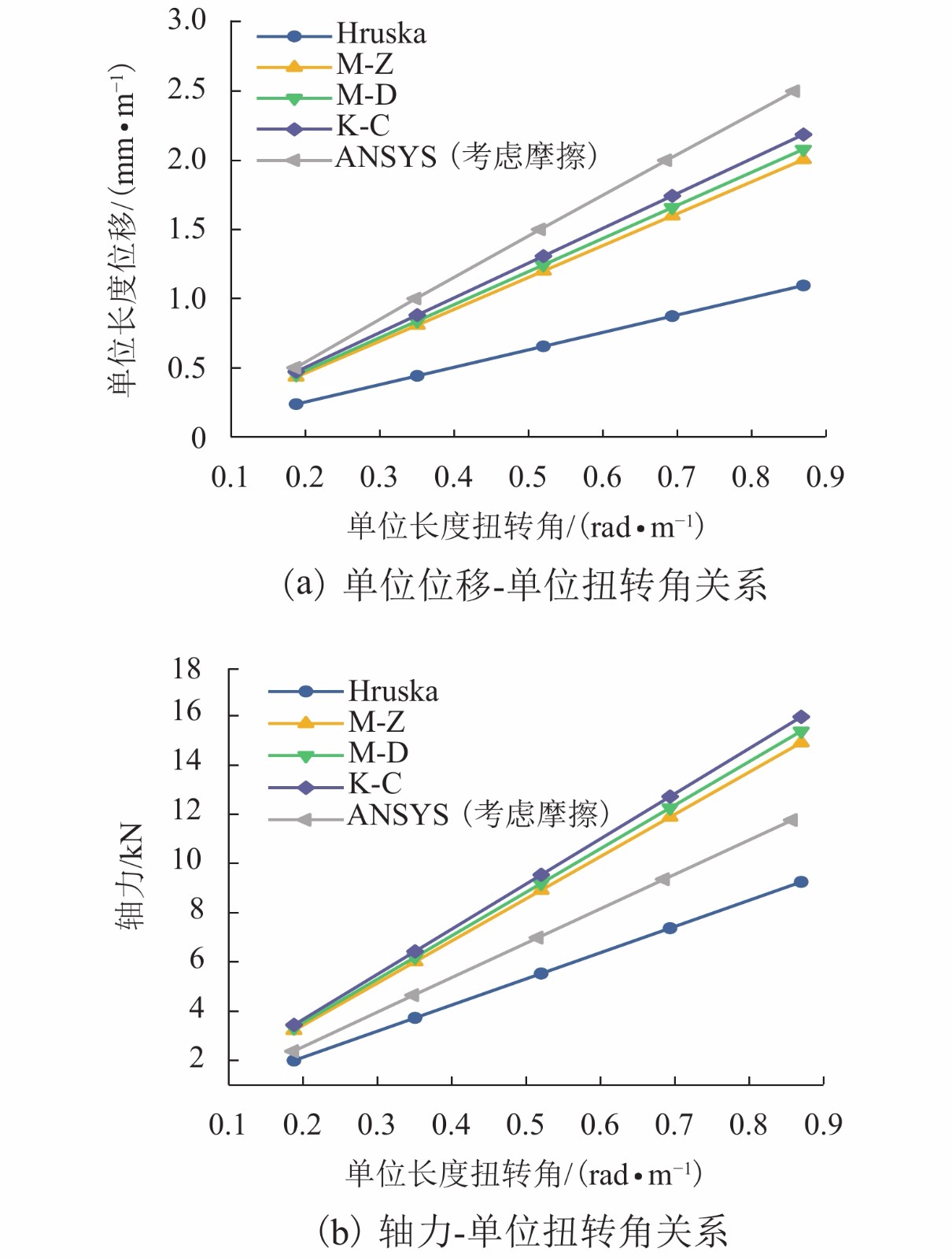
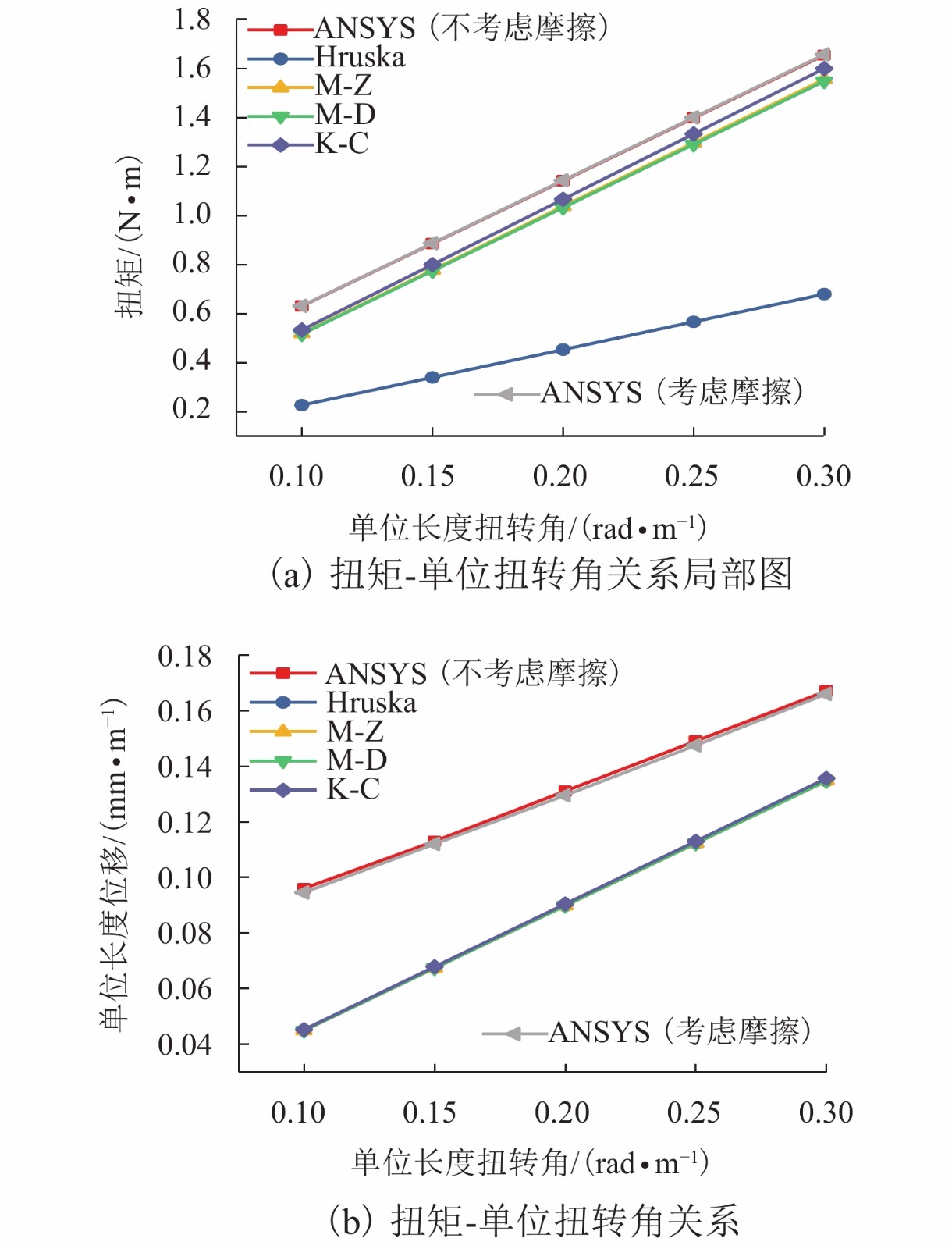
方法 Fε/kN Mϕ/
(N•m2•rad−1)Fϕ/
(N•m)Mε/
(N•m)ANSYS
仿真5 429.92 6.138 2 101.98 2 255.77 Hruska 6 224.01 3.517 2 792.47 2 792.47 M-Z 6 224.01 6.436 2 792.47 2 792.47 M-D 6 224.01 6.349 2 792.47 2 655.59 K-C 6 183.53 6.496 2 792.47 2 583.80 表 5 各方法精度对比
Table 5. Accuracy comparison of methods
方法 剪切模量/GPa 与试验误差/% ANSYS仿真 4.572 3.79 郭应龙等[4] 2.934 33.39 Hruska 2.012 54.33 M-Z 4.606 4.56 M-D 4.583 4.04 K-C 4.736 7.51 扭转试验 4.405 -
晏致涛,黄静文,李正良. 基于结点6自由度的分裂导线有限元模型[J]. 工程力学,2012,29(8): 325-332. doi: 10.6052/j.issn.1000-4750.2010.08.0626YAN Zhitao, HUANG Jingwen, LI Zhengliang. Finite element model of split conductor based on 6-DOF node[J]. Engineering mechanics, 2012, 29(8): 325-332. doi: 10.6052/j.issn.1000-4750.2010.08.0626 谢增,刘吉轩,刘超群,等. 架空输电线路分裂导线扭转刚度计算新方法[J]. 西安交通大学学报,2012,46(2): 100-105.XIE Zeng, LIU Jixuan, LIU Chaoqun, et al. A new method for calculating torsional stiffness of split conductor of overhead transmission line[J]. Journal of Xi’an Jiaotong University, 2012, 46(2): 100-105. 中华人民共和国国家质量监督检验检疫总局, 中国国家标准化管理委员会. 圆线同心绞架空导线: GB/T 1179—2017 [S]. 北京: 中国标准出版社, 2017. 郭应龙, 李国兴, 尤传永. 输电线路舞动[M]. 北京: 中国电力出版社, 2003: 131-140. SUSLOV B M. On the modulus of wire ropes[J]. Wire and Wire Products, 1936, 111: 176-182. HRUSKA F H. Calculations of stresses in wire rope[J]. Wire and Wire Products, 1951, 26: 766-767,799-801. LANTEIGNE J. Theoretical estimation of the response of helically armored cables to tension,torsion,and bending[J]. Journal of Applied Mechanics, 1985, 52(2): 423-432. doi: 10.1115/1.3169064 MACHIDA S, DURELLI A J. Response of a strand to axial and torsional displacements[J]. Journal of Mechanical Engineering Science, 1973, 15(4): 241-251. doi: 10.1243/JMES_JOUR_1973_015_045_02 MC CONNELL K G, ZEMKE W P. A model to predict the coupled axial torsion properties of ACSR electrical conductors[J]. Experimental Mechanics, 1982, 22(7): 237-244. doi: 10.1007/BF02326388 KNAPP R H. Derivation of a new stiffness matrix for helically armoured cables considering tension and torsion[J]. International Journal for Numerical Methods in Engineering, 1979, 14(4): 515-529. doi: 10.1002/nme.1620140405 COSTELLO G A, PHILLIPS J W. Effective modulus of twisted wire cables[J]. Journal of the Engineering Mechanics Division, 1976, 102(1): 171-181. doi: 10.1061/JMCEA3.0002092 LOVE B A E H. A treatise on the mathematical theory of elasticity[M]. [S.l.]: Dover Publications, 1944. KUMAR K. S, Cochran J E. Closed-form analysis for elastic deformations of multilayered strands[J]. Journal of Applied Mechanics, 1987, 54(4): 898. doi: 10.1115/1.3173136 XIANG L, WANG H Y, CHEN Y, et al. Elastic-plastic modeling of metallic strands and wire ropes under axial tension and torsion loads[J]. International Journal of Solids and Structures, 2017, 129: 103-118. doi: 10.1016/j.ijsolstr.2017.09.008 JOLICOEUR C, CARDOU A. A numerical comparison of current mathematical models of twisted wire cables under axisymmetric loads[J]. Journal of Energy Resources Technology, 1991, 113(4): 241-249. doi: 10.1115/1.2905907 RAOOF M, KRAINCANIC I. Critical examination of various approaches used for analyzing helical cables[J]. The Journal of Strain Analysis for Engineering Design, 1994, 29(1): 43-55. doi: 10.1243/03093247V291043 CHEN Y, MENG F, GONG X. Full contact analysis of wire rope strand subjected to varying loads based on semi-analytical method[J]. International Journal of Solids and Structures, 2017, 117: 51-66. doi: 10.1016/j.ijsolstr.2017.04.004 张秋桦. 张拉导线应力及刚度的研究[D]. 北京: 华北电力大学, 2017. 杨光甫. 钢芯铝绞线等效弯曲刚度研究[D]. 北京: 华北电力大学, 2015. 卢银均,刘闯,孟遂民. 钢芯铝绞线弯曲状态受力分析[J]. 三峡大学学报(自然科学版),2018,40(3): 66-69.LU Yinjun, LIU Chuang, MENG Suimin. Stress analysis of steel cored aluminum strand in bending state[J]. Journal of Three Gorges University (Natural Science), 2018, 40(3): 66-69. UTTING W S, JONES N. The response of wire rope strands to axial tensile loads—part I:experimental results and theoretical predictions[J]. International Journal of Mechanical Sciences, 1987, 29(9): 605-619. doi: 10.1016/0020-7403(87)90033-6 -






 下载:
下载: