Optimal Selection of Mountain Railway Location Design Based on Twice-Improved TOPSIS Method
-
摘要:
为克服传统TOPSIS(逼近理想解排序法)在山区铁路线路方案比选中不能排除指标间相关性干扰、易使决策工作复杂化的缺点,同时为能充分考虑决策工作的不确定性特征,首先,以马氏距离代替传统TOPSIS中的欧氏距离,实现第一次改进;接着,以相关系数矩阵代替马氏距离中的协方差矩阵,实现第二次改进;然后,运用语言类模糊数、区间数、云模型等实现定性指标的定量化,并以二次改进的TOPSIS为基础构建山区铁路线路方案综合比选模型;最后,研究了综合比选模型在某山区铁路巴塘至昌都段局部线路走向方案比选中的应用. 研究结果表明:二次改进的TOPSIS能有效排除决策工作中指标间的相关性干扰,简化决策过程;云模型的运用能有效克服决策方法在处理定性语言不确定性方面的不足;线路方案综合比选模型的应用研究结果与该山区铁路工程预可研中的推荐结果一致,即比选结果均为经白玉、江达的线路方案,故该模型可作为今后线路方案比选工作中一种新的应对途经.
Abstract:This work aims to overcome the shortcomings of the traditional TOPSIS (technique for order of preference by similarity to an ideal solution) method, which cannot exclude the interference from correlation among indexes that can easily complicate decision-making operations during optimal selection of mountain railway location designs, and thus enable full consideration of any uncertain characteristics during decision-making. First, the Mahalanobis distance was used to replace the Euclidean distance in traditional TOPSIS, thus achieving the first improvement in TOPSIS. Then, the correlation coefficient matrix was used to replace the covariance matrix in the Mahalanobis distance, which represents the second improvement in the TOPSIS method. Then, linguistic fuzzy numbers, interval numbers, and a cloud model were used to achieve quantification of the qualitative indexes, and a comprehensive optimization model for mountain railway location design was constructed based on the twice-improved TOPSIS method. Finally, the comprehensive optimization model was applied to the partial route direction scheme of the Batang to Changdu section of a specific mountain railway. The results show that the twice-improved TOPSIS method can effectively exclude the interference from the correlation among indexes and thus simplifies the decision-making process. In addition, application of the cloud model can overcome the deficiency in the decision-making method with regard to its ability to deal with the uncertainty of qualitative language. The application results from the comprehensive optimization model coincide with the recommended results of the pre-feasibility study of the mountain railway, i.e., the optimization results from both approaches lead to the route scheme passing through Baiyu and Jiangda, thus indicating that the proposed model can be used as a new approach for route direction optimization in future mountain railway location designs.
-
Key words:
- mountain railway /
- scheme optimization /
- twice-improved method /
- uncertainty characteristic /
- cloud model /
- TOPSIS
-
有许多山区铁路项目已纳入规划或是正在建设,山区干线铁路、高速铁路等的规划与建设将为我国优化干线铁路网布局,推进高速铁路“八纵八横”主通道建设,拓展铁路网覆盖范围以及拉动山区经济增长打下坚实基础.
山区地形困难、地质复杂、生态脆弱且城镇经济据点稀少,因此山区铁路线路方案比选更加注重工程的安全性、地质选线原则、绿色环保理念以及对沿线经济发展的带动效果等[1]. 在此之前,国内已有众多学者就山区铁路线路方案比选做了大量研究:罗圆[2]将铁路选线视为带有不确定性的多目标决策过程,分别将可靠性数学理论、风险分析理论和效用理论等运用于山区铁路线路方案评价,提出基于不确定性分析的山区铁路线路方案评价方法;邓汉轩[3]突出山区铁路线路方案优选过程中的环境因素,在运用西部山区铁路选线工程实例的基础上,比较模糊综合评价和人工神经网络评价两种方法,最终得出人工神经网络评价法的效果更好;林凯[4]在进行困难艰险山区高速铁路线路方案综合优化研究时,将防灾救援部分考虑进去,建立考虑防灾救援的困难艰险山区高铁线路方案综合优化模型;张明威[5]分析了多岭和强震山区的铁路建设风险,归纳出强震山区越岭铁路的选线策略,同时结合层次分析法与熵权法,构建强震山区越岭段铁路线路方案风险评价模型.
TOPSIS (technique for order of preference by similarity to an ideal solution)是由Hwang和Yoon在1981年提出的一种用于解决单一型或混合型多属性决策问题的方法[6],其主要优点是便于理解、计算较简单、适用范围广、几何意义直观等. 但TOPSIS有两个较为突出的不足之处:无法消除决策问题中属性指标间的相关性影响;计算虽简单,但需额外的规范化和赋权手段来分别解决属性指标量纲不一致和权重分配的问题,这不免会使决策过程复杂化. 而且当采用主观赋权手段时,决策结果的可信度将降低,当采用主客组合赋权手段时,决策过程将进一步复杂化. 近年来,国内外学者对TOPSIS的改进研究非常多样化:Jahanshahloo等[7]运用区间数来改进TOPSIS,并提出一种基于区间数的TOPSIS决策问题扩展算法;Dymova等[8]采用区间2型模糊值的alpha分割来弥补TOPSIS在进行2型模糊扩展时的不足,且经实例证明此2型模糊扩展不受已知方法的限制;王先甲等[9]以马氏距离代替欧氏距离来改进TOPSIS,最终达到消除变量间相关性干扰的目的;Wang等[10]将基于五级法改进的AHP (analytic hierarchy process)作为TOPSIS的额外赋权工具,由此构建油气管道客观风险综合评估模型.
就多数对TOPSIS的改进研究而言,一些将改进重点放在决策环境的拓展上,一些注重对赋权方式的完善,另一些从属性指标中的不确定性信息着手加以改进,还有一些就方法本身的单个缺陷做相应改进,但鲜有学者同时兼顾上述4个方面,并且弥补TOPSIS的两个不足. 本文先以马氏距离代替TOPSIS中的欧氏距离,以相关系数矩阵代替马氏距离中的协方差矩阵,实现TOPSIS的二次改进,既弥补了TOPSIS的两个不足,完成对属性指标的客观赋权,又解决了协方差可能导致的问题;然后运用区间数和云模型实现从定性语言到定量表示的过渡,既充分考虑了属性指标的不确定性和离散性,又很好地克服了评价方法在处理模糊性和随机性方面的不足;最后将上述方法及模型应用于山区铁路选线决策环境,决策结果表明该方法及模型科学、有效,可为日后解决类似决策问题提供一定的参考.
1. TOPSIS及其改进分析
1.1 TOPSIS的步骤
假设有m个备选方案A1、A2、···、Am和n个属性指标C1、C2、···、Cn. xij为Ai在Cj下的指标值(i=1,2,···,m; j=1,2,···,n). TOPSIS的步骤如下:
步骤1 根据属性指标值构造的决策矩阵为
X = [x11x12⋯x1nx21x22⋯x2n⋮⋮⋮xm1xm2⋯xmn]. (1) 步骤2 进行规范化处理,消除决策矩阵中数据量纲不同带来的影响,处理后得到的标准化矩阵为
R = [r11r12⋯r1nr21r22⋯r2n⋮⋮⋮rm1rm2⋯rmn]. 步骤3 确定权重W=diag(w1,w2,···,wi,···,wn),权重满足wi > 0,
n∑i=1wi = 1 ,W与标准化矩阵相乘,得到的价值矩阵为V = RW = [v11v12⋯v1nv21v22⋯v2n⋮⋮⋮vm1vm2⋯vmn]. (2) 步骤4 确定正理想方案S + 和负理想方案S−:
S + ={s + 1,s + 2,⋯,s + n} ,S−={s−1,s−2,⋯,s−n} . 求解方法如下:当Cj为效益型指标时:
{s+j = max 当Cj为成本型指标时:
\left\{ \begin{gathered} s_{{j}}^ + {\text{ = }}\min \left\{ {{v_{{{ij}}}}\left| {1 \leqslant {{i}} \leqslant {{m}}} \right.} \right\} ,\hfill \\ s_{{j}}^ - {\text{ = }}\max \left\{ {{v_{{{ij}}}}\left| {1 \leqslant {{i}} \leqslant {{m}}} \right.} \right\} .\hfill \\ \end{gathered} \right.\tag{3b} 当无步骤2和步骤3时,将式(2)、(3)中的vij替换成xij即可.
步骤5 分别计算Ai到S + 的欧氏距离Di + ,Ai到S− 的欧氏距离Di−. 如式(4).
\left\{ { \begin{array}{l} D_{{i}}^ + {\text{ = }}\sqrt {\displaystyle\sum\limits_{{{j = }}1}^{{n}} {{{\left( {{v_{{{ij}}}} - s_{{j}}^ + } \right)}^2}} },\\ D_{{i}}^ - {\text{ = }}\sqrt {\displaystyle\sum\limits_{{{j = }}1}^{{n}} {{{\left( {{v_{{{ij}}}} - s_{{j}}^ - } \right)}^2}} }. \end{array} } \right. (4) 步骤6 计算各备选方案的相对贴近度. 求解方法如下:
C_{{i}}^ * {\text{ = }}\dfrac{{D_{{i}}^ - }}{{D_{{i}}^ + + D_{{i}}^ - }}, \tag{5a} (5) C_{{i}}^ * {\text{ = }}\dfrac{{D_{{i}}^ + }}{{D_{{i}}^ + + D_{{i}}^ - }}. \tag{5b} (5) 步骤7 根据Ci*的大小进行相对优劣排序,显然Ci*∈[0,1.0]. 对式(5a),Ci* 越大越优;对式(5b),Ci* 越小越优.
1.2 TOPSIS第一次改进
1.2.1 TOPSIS的局限性分析
1) 由于欧式距离本身的局限性,当指标间存在相关性,指标所包含的信息重叠,欧式距离失效,决策结果不准确. 而现实决策问题的属性指标几乎都存在相关性,所以这一问题必须得到解决.
2) 属性指标的量纲通常不一致,TOPSIS会因量纲不一致而失效,而欧氏距离不能消除量纲不一致带来的影响,需要借助额外的规范化手段来消除,使决策过程复杂化. 1.1节中的步骤3是各属性指标的赋权过程,需要借助额外的赋权手段才能完成,如果采用主观赋权手段确定权重,决策结果的可信度会降低,如果采用主客组合赋权手段确定权重,由于组合赋权过程本身就很繁复,决策过程将进一步复杂化. 因此,上述两个方面是亟须改进的[11].
1.2.2 TOPSIS的改进思路
马氏距离是由印度统计学家Mahalanobis在1936年提出的一种统计距离. 相对于欧氏距离,马氏距离对属性指标的变化敏感,独立于测量尺度,更能体现各状态或特征间的关系,并且还能排除指标间不同程度的相关性干扰. 同时马氏距离是以标准差函数计算指标权重的,指标值变化大的内化权重赋值小,克服了在计算距离时指标变化程度不同但起相同作用的缺点.
本文利用马氏距离本身能有效排除指标间的相关性干扰、不受量纲影响以及内化于公式的客观赋权方式的优良特性,以马氏距离代替欧氏距离来实现TOPSIS的第一次改进. 改进后的TOPSIS不仅可以排除指标间的相关性干扰,而且可以省略1.1节中的步骤2和步骤3.
1.3 TOPSIS第二次改进
1.3.1 马氏距离的局限性分析
马氏距离考虑的是属性指标间的协方差,协方差是依赖量纲的量,且其大小代表的是两属性对各自均值偏差的综合程度,故协方差可能导致计算结果不稳定,极易放大属性间的影响,不能准确代表属性间的关联程度的问题[12]. 马氏距离在TOPSIS中的表达式为
\left\{ { \begin{array}{l} D({A}_{i},{S}^{ + })\text=\sqrt{\left({X}_{i}-{S}^{ + }\right){{\boldsymbol{\varSigma}} }^{-1}{\left({X}_{i}-{S}^{ + }\right)}^{\text{T}}}, \\ D({A}_{i},{S}^-)\text=\sqrt{\left({X}_{i}-{S}^-\right){{\boldsymbol{\varSigma}} }^{-1}{\left({X}_{i}-{S}^-\right)}^{\text{T}}}, \end{array}} \right. (6) 式中:D(Ai,S + )和D(Ai,
{S^ - } )分别为备选方案Ai到正理想方案S + 与负理想方案{S^ - } 的马氏距离;Σ为决策矩阵X的协方差矩阵,Σ −1 为协方差矩阵的逆矩阵,Xi=[xi1 xi2 ··· xin].1.3.2 TOPSIS的改进思路
任意两个随机变量x、y的相关系数ρxy与协方差cov(x,y)的根本区别在于,当描述其关联程度时前者不受量纲的影响,后者受量纲的影响,而且前者的描述过程更稳定,结果更准确[13]. 因此,以相关系数矩阵
\boldsymbol{Q} 代替协方差矩阵Σ来改进马氏距离,能解决协方差可能导致的问题. 将对马氏距离的改进作为对TOPSIS的第二次改进,再次改进后的TOPSIS相比第一次改进更具科学性和有效性.2. 方案比选指标探讨
2.1 指标选用
山区铁路线路方案比选需要考虑的指标多而涉及面广,指标选用时的侧重点和标准均有所不同. 本文确定指标体系的递阶结构由总目标层、宏观目标层和微观目标层组成,在总结众多其他学者研究成果的基础上,秉承系统性、实用性以及定性与定量相结合等指标体系构建原则,从山区铁路线路方案与技术经济条件、周遭环境以及社会意义等大系统的协调发展出发,构建如表1所示的山区铁路线路方案比选指标体系. 表1中的指标主要用于原则方案的比选,在进行实际方案比选时,可根据具体情况选择相应的指标重新构建指标体系[14-15].
2.2 定性指标处理
山区铁路线路方案比选指标体系中既有定量指标,又有定性指标. 定量指标直接采用精确数量化,定性指标的常用量化方式有区间数、三角模糊数和云模型,其中区间数只能部分考虑定性语言的不确定性特征,三角模糊数中3个变量的权重值相等,故其并未体现出变量的重要性. 本文先采用语言类模糊数表示定性指标,然后采用区间数作为语言类模糊数的转化形式,最后运用云模型将区间数转化为精确数,该精确数即作为定性指标值.
2.2.1 区间数
Moore在1965年首次提出区间数的概念. 区间数的获得无需较多的假设和先验知识,能便捷地表示数据中的不确定信息,而且在求解不确定性问题时能减少主观因素的影响. 当某个问题的原始特征存在不确定性,且在给定的范围内时,就可以用区间数来表示[16].
a=\left[{a}^{\text{L}},{a}^{\text{U}}\right]=\left\{x|0 < {a}^{\text{L}}\,\leqslant\, x\,\leqslant\, {a}^{\text{U}},{a}^{\text{L}},{a}^{\text{U}}\in {\bf{R}}\right\}\; 为一个区间数. 当aL = aU时,区间数退化为普通实数,aL和aU分别为区间数的下界和上界[17].S={很好,好,较好,一般,较差,差,很差}或{很大,大,较大,一般,较小,小,很小}为语言类模糊数集. 定性语言“很好或很大”即为语言类模糊数,其本身存在一定的不确定性特征[17]. 区间数与语言类模糊数的转化关系如表2所示.
表 1 山区铁路线路方案比选指标体系Table 1. Index system of optimal selection of mountain railway location design总目
标层宏观目标层 微观目标层 山区铁路线路方案比选 技术可行性 线路长度
输送能力
通过能力
最小曲线半径
展线系数
桥隧总长
桥隧占比
工程工期
最大坡度
拔起高度
土石方工程量
征地量
拆迁建筑物数量经济合理性 土建工程投资费
与列车有关的运营费
固定设备维修费
机车车辆购置费
预备费及其他费用
年换算工程运营费
净现值
投资回收期
内部收益率施工条件及环境影响 工程对自然生态环境的影响
工程对历史文化名胜的影响
噪音对沿线居民的影响
江河湖泊等对工程的影响
不良地质条件对工程的影响社会经济政治意义 促进周边地区经济发展的能力
吸引客货流的能力
改善路网布局的意义
满足地方需求的能力
对城市规划布局的影响表 2 语言类模糊数与区间数的转化Table 2. Conversion between linguistic fuzzy number and interval number语言类模糊数 区间数 很差或很小 [0,1.0] 差或小 [1.0,3.0] 较差或较小 [3.0,4.5] 一般 [4.5,5.5] 较好或较大 [5.5,7.0] 好或大 [7.0,9.0] 很好或很大 [9.0,10.0] 2.2.2 云模型概述
云模型是由李德毅院士以概率统计和模糊数学为基础提出的一种定性语言与定量表示间不确定性的转换模型. 云模型不仅能充分考虑定性语言的不确定性和离散性,实现其与定量数值间的相互转换,而且克服了评价方法在处理模糊性和随机性方面的不足. 正态云模型是最基本也是最常用的云模型[18]. 设
\mu \left(x\right):U\to \left[0,1.0\right]\text{,}\forall x\in X\left(X\subseteq U\right)\text{,}x\to \mu \left(x\right), (7) 为论域U中的任意一个元素x对定性概念T的隶属度,云即μ(x)在U上的分布. 其中,x为云滴,是云的最基本组成元素,也是定性概念T的一个量化值.
每个定性概念都可以用云的期望Ex、熵En与超熵He来定量表示,这3个数值称为云模型的数字特征,记作C(Ex,En,He). 其中:期望Ex表示定性语言量化为云滴群的中心点,是最能代表某一定性语言的取值;熵En反映了云滴群分布的不确定性;超熵He表示定性语言量化为云滴群的离散性[19].
2.2.3 正向云、条件云发生器
云模型发生器包括正向云发生器、逆向云发生器和条件云发生器. 正向云发生器是由定性语言向定量数值转换的映射,即对定性语言的量化算法. 正向云发生器输入的是需要转换的定性语言的3个数字特征,输出的是
n 个云滴——{\rm{drop}}\left({x}_{{t}},{\mu }_{{t}}\right) . 正向云发生器算法如下:步骤1 生成正态随机数
{E}{'}_{{n}_{{t}}}\text{=}{\rm{NORMRND}} ({E}_{n}, {\left({H}_{e}\right)}^{2}) ,其中{E_n} 和{\left( {{H_e}} \right)^2} 分别为期望和方差;步骤2 生成正态随机数
{x}_{{t}}\text{=}{\rm{NORMRND}} \bigg({E}_{x},\bigg. \left.{\left({E}{'}_{{n}_{{t}}}\right)}^{2}\right) ,其中\left({E}{'}_{{n}_{{t}}}\right) 为方差;步骤3 计算
{\mu _{{t}}}{\text{ = }}\exp \left( - \dfrac{{{{\left( {{x_{{t}}} - {E_x}} \right)}^2}}}{2{\left({E}{'}_{{n}_{{t}}}\right)}^{2}}\right) ,\left({x}_{{t}},{\mu }_{{t}}\right) 即为数域中任意一个云滴;步骤4 重复前面3个步骤,直到产生满足条件的云滴或者设定的
n 个云滴为止.条件云发生器分为
X 条件云发生器和Y 条件云发生器,均以云模型的不确定性推理为基础,结合正向云发生器实现由定性语言向定量数值的转换. 由于通过发生器产生的云滴与输出值均不唯一,也即不确定,故形成不确定性推理. 当定性规则描述成“如果C1,那么C2”,则C1和C2均为U中的定性概念,也是不确定性推理规则的前件云与后件云,该定性规则即为单条不确定性推理规则. 不确定性推理算法如下:输入:前件云
{C_1} 的期望{E_{{x_{{C1}}}}} 、熵{E_{{n_{{C1}}}}} 、超熵{H_{{e_{{C1}}}}} 和特定数值{x_0} 以及后件云{C_2} 的期望{E_{{x_{{C2}}}}} 、熵{E_{{n_{{C2}}}}} 、超熵{H_{{e_{{C2}}}}} . 输出:对应于前件云生成确定度为{\mu _{{t}}} 的后件云定性语言的定量数值y01. 具体步骤为步骤1 由
X 条件云发生器生成正态随机数{E}{'}_{{C}_{1}}\text{=}{\rm{NORMRND}}\left({E}_{{n}_{{C1}}},{\left({H}_{{e}_{{C1}}}\right)}^{2}\right) ,其中{E_{{n_{{C1}}}}} 和{\left( {{H_{{e_{{C1}}}}}} \right)^2} 分别为期望和方差;步骤2 由
X 条件云发生器计算{x_0} 在定性语言{C_1} 上的确定度,{\mu _t} = \exp \left( { - \dfrac{{\left( {{x_0} - {E_{{x_{{C1}}}}}} \right)}}{{2\left( {E{'}_{{c_1}}} \right)}}} \right) ;步骤3 由
Y 条件云发生器生成正态随机数{E}{'}_{{C}_{2}}\text{=}{\rm{NORMRND}}\left({E}_{{n}_{{C2}}},{\left({H}_{{e}_{{C2}}}\right)}^{2}\right) ;步骤4 在
Y 条件云发生器内,如果{x_0} 激活{C_1} 的上升沿,则{C_2} 用上升沿计算y0. 即当{x_0} \leqslant {E_{{x_{{C1}}}}} 时,{y_0}={E_{{x_{{C2}}}}} - E{'}_{{C_2}} \sqrt { - 2{\text{ln}}\;{\mu _{{t}}}} ;步骤5 在
Y 条件云发生器内,如果{x_0} 激活{C_1} 的下降沿,则{C_2} 用下降沿计算y0. 即当{x_0} > {E_{{x_{{C1}}}}} 时,{y_0}{\text{ = }}{E_{{x_{{C2}}}}} + E{'}_{{C_2}} \sqrt { - 2{\text{ln}}\;{\mu _{{t}}}} .3. 基于二次改进的TOPSIS的山区铁路线路方案比选步骤
步骤1 确定参与比选的m个山区铁路线路方案A1,A2,···,Am和n个属性指标C1,C2,···,Cn中,Cj (j=1,2,···,k)为定量指标,Cj (j=k + 1,···,n)为定性指标. xij为Ai在Cj下的指标值,定量指标值取用各方案提供的精确数.
步骤2 先聘请专家用语言类模糊数给定性指标赋值;接着对照表2选取相应的区间数;然后由式(8)将区间数转化为云模型的数字特征(云模型数),如表3所示,其中7个云模型数为不确定性推理算法中前件云的定量表示;最后采用百分制数值来表征定性指标因子水平的高低,水平越高,因子分值就越大. 分值大小的定性评语与相应的百分制云模型描述如表4所示,其中7个云模型描述为不确定性推理算法中后件云的定量表示.
\left\{ \begin{gathered} {E_x}{\text{ = }}\left( {{a^{\text{U}}} + {a^{\text{L}}}} \right)/2 ,\hfill \\ {E_n}{\text{ = }}\left( {{a^{\text{U}}} - {a^{\text{L}}}} \right)/6 ,\hfill \\ {H_e}{\text{ = }}c ,\hfill \\ \end{gathered} \right. (8) 式中:c为常数,与语言类模糊数的本身离散程度和模糊度有关,一般取0.02.
以定性指标“改善路网布局的意义”为例,由专家知识构建语言类模糊数、定性评语和云模型描述间的不确定性推理规则. 当改善路网布局的意义“很小”,则定性评语为“很低”,云模型描述为(10,5/3,0.02);当改善路网布局的意义“小”,则定性评语为“低”,云模型描述为(30,5/3,0.02). 以此类推.
步骤3 运用云模型发生器获得由精确数表示的定性指标值,具体操作如下:
表 3 语言类模糊数、区间数与云模型数的转化Table 3. Conversion among linguistic fuzzy number, interval number and cloud model number语言类模糊数 区间数 云模型数 很差或很小 [0,1.0] (1/2,1/6,0.02) 差或小 [1.0,3.0] (2,1/3,0.02) 较差或较小 [3.0,4.5] (7.5/2,1.5/6,0.02) 一般 [4.5,5.5] (5,1/6,0.02) 较好或较大 [5.5,7.0] (12.5/2,1.5/6,0.02) 好或大 [7.0,9.0] (8,1/3,0.02) 很好或很大 [9.0,10.0] (9.5,1/6,0.02) 表 4 定性评语与云模型描述的转化Table 4. Conversion between qualitative comment and cloud model description定性评语 云模型描述 很低 (10,5/3,0.02) 低 (30,5/3,0.02) 较低 (45,5/3,0.02) 中等 (55,5/3,0.02) 较高 (65,5/3,0.02) 高 (80,5/3,0.02) 很高 (90,5/3,0.02) 首先由表3中的7个云模型数驱动正向云发生器,将生成的7个正态随机数
{x_{\text{t}}} 的均值作为X 条件云发生器的特定数值{x_0} ;随后将{x_0} 和实际方案{A_{\text{i}}} 的某一定性指标的对应云模型数代入X 条件云发生器,即执行2.2.3节中不确定性推理算法的步骤1、2,得到相应的确定度{\mu _{{t}}} ;最后将{\mu _{{t}}} 和通过不确定性推理规则确定的云模型描述作为Y 条件云发生器的输入,激活后随机产生云滴{\rm{drop}}\left({y}_{0},{\mu }_{{t}}\right) ,即执行不确定性推理算法的步骤3、4或5,精确数{y_0} 即作为该定性指标的取值.步骤4 由上述步骤确定的所有指标值构造方案比选的决策矩阵,如式(1).
步骤5 根据二次改进的TOPSIS求得相对贴近度,并根据相对贴近度的大小进行方案的相对优劣排序. 对式(9a),
C_{{i}}^ {*} 越大越优;对式(9b),C_{{i}}^ {*} 越小越优.{C}_{{i}}^{\ast }\text=\dfrac{D({A}_{{i}},{S}^-)}{D({A}_{{i}},{S}^{ + }) + D({A}_{{i}},{S}^-)} ,\tag{9a} (9) {C}_{{i}}^{\ast }\text=\dfrac{D({A}_{{i}},{S}^{ + })}{D({A}_{{i}},{S}^{ + }) + D({A}_{{i}},{S}^-)} . \tag{9b} (9) 4. 实例分析
结合某山区铁路巴塘至昌都段局部线路走向方案,对比分析由本文方法及模型得到的比选结果与实际工程的优选结果,以说明该方法及模型的科学性与有效性.
该铁路巴塘至昌都段位于横断山脉中部,线路起于海子山西麓,在跨金沙江、穿芒康山脉、跨澜沧江后,止于昌都市. 区域交通主要由G214、G317、S501、巴白路等组成. 沿线政治经济据点坐落不一,交通状况错综,地形地质复杂,自然环境自我修复能力较弱. 根据沿线政治经济据点分布、交通状况以及地形地质条件等因素,研究了经白玉、江达,经白玉、贡觉、江达,经白玉、贡觉,经贡觉、江达和经贡觉取直五大线路走向方案.
通过综合分析,选用表5所示的9个指标进行线路方案综合比选(取值根据该山区铁路的预可行性研究报告的相关内容确定). 其中:A1~A5依次代表经白玉、江达,经白玉、贡觉、江达,经白玉、贡觉,经贡觉、江达和经贡觉取直五大线路走向方案;C1~C9依次为线路长度、桥隧总长、工程静态投资、年换算工程运营费、对自然生态环境的影响、不良地质条件对工程的影响、促进周边经济发展的能力、改善路网布局的意义、满足地方需求的能力9个指标,前6个为成本型指标,后3个为效益型指标.
运用正向云发生器、条件云发生器以及不确定性推理机制,将给定性指标赋值的语言类模糊数转化为百分制数值,也即获得由精确数表示的定性指标值. 运算过程由MATLAB实现,结果如表6所示.
由5个方案的所有指标值构造方案比选的决策矩阵X,并确定正理想方案
{S^ + } 和负理想方案{S^ - } ,{S^ + } ={204.711,188.375,254.251,318.326,55.0107,8.7623,88.7622,88.7558,88.7634};{S^ - } ={274.562,242.171,330.298,416.236,81.6858,55.0107,45.5362,55.0107,64.4946}由式(9a)计算5个方案的相对贴近度,结果为C1*=0.506 73,C2*=0.453 37,C3*=0.452 36,C4*=0.263 36,C5*=0.383 28
式(9a)计算值越大,则方案越优,因此不难得出方案A1最优. 对表5中的指标进行分析可知,虽然经白玉、江达方案线路长度较长,工程投资较多,但地质条件、交通条件、设站条件最好,而且该方案经过了玉龙铜矿,同时辐射范围最广、能更好地带动地方经济发展,满足地方意见及其他要求. 如以所选择的9个指标作为线路方案综合比选的主要依据,则由本文方法及模型得到的比选结果与该项目的预可行性研究报告中建议选择的结果较为一致,说明该方法及模型具有较好的科学性与有效性.
表 5 线路走向方案及其属性指标取值Table 5. Route direction scheme and its attribute index value指标 A1 A2 A3 A4 A5 C1/km 274.562 267.808 243.229 234.100 204.711 C2/km 242.171 222.648 209.870 203.772 188.375 C3/亿元 330.298 324.048 297.469 287.943 254.251 C4/亿元 416.236 407.872 373.600 361.216 318.326 C5 小 一般 较小 一般 小 C6 一般 较大 大 大 很大 C7 很好 好 较差 一般 较差 C8 很大 较大 一般 较大 一般 C9 很好 很好 较好 较好 较好 表 6 由精确数表示的定性指标值Table 6. Qualitative index value represented by exact number方案 C5 C6 C7 C8 C9 A1 81.685 8 55.010 7 88.762 2 88.755 8 88.763 4 A2 55.010 7 44.495 9 78.354 8 64.495 7 88.760 5 A3 65.538 2 28.356 3 45.537 2 55.010 7 64.495 2 A4 55.010 7 28.355 4 55.010 8 64.494 7 64.494 6 A5 81.683 1 8.762 3 45.536 2 55.010 7 64.496 3 5. 结 论
1) 现实决策问题的指标间几乎都会存在相关性,指标的量纲会有差别,指标的赋权过程或不够科学或过于复杂,这要么使TOPSIS的决策过程复杂化,要么使TOPSIS的决策结果不够准确. 鉴于马氏距离本身带有能有效排除指标间相关性干扰等优良特性,以马氏距离代替欧氏距离来改进TOPSIS,改进后的TOPSIS既能保证决策结果的科学性与准确性,又能使决策过程简单化.
2) 马氏距离在TOPSIS中的表达要考虑协方差. 在计算马氏距离时,可能会因考虑协方差而导致不能准确代表属性间的关联程度等问题的出现. 任意两变量的相关系数能够弥补协方差的不足,能准确地描述变量间的关联程度,而且描述过程稳定,描述结果准确. 故以相关系数矩阵代替协方差矩阵来改进马氏距离,把对马氏距离的改进作为TOPSIS的第二次改进,再次改进后的TOPSIS更加科学、客观.
3) 语言类模糊数本身带有一定的不确定性特征. 区间数的运算过程虽简单、直观,但其不足之处在于不能充分考虑定性语言的不确定性特征. 而云模型既能充分考虑定性语言的不确定性和离散性,又克服了评价方法在处理模糊性和随机性方面的不足,还能实现由定性语言向定量表示的有效转换. 所以本文选用云模型实现语言类模糊数的精确数化.
4) 针对二次改进的TOPSIS,在求算马氏距离时需计算相关系数矩阵的逆. 本文实例分析中的相关系数矩阵存在逆矩阵. 但在极少数情况下,当属性指标线性强相关时,相应的相关系数矩阵可能不可逆,此时马氏距离将无法求算,进而无法得到决策结果. 要求相关系数矩阵一定可逆不太现实,但如何确保在推进决策工作时能求算出相关系数矩阵的“逆矩阵”是值得进一步研究的. 例如当相关系数矩阵不可逆时,可采用奇异值分解法求算其正定的“广义”逆矩阵,以其代之.
5) 结合某山区铁路巴塘至昌都段局部线路走向方案,由本文方法及模型得到的比选结果同实际工程的优选结果基本一致,说明该方法及模型具有较好的科学性与有效性,并为今后类似决策问题的解答提供一定的参考.
-
表 1 山区铁路线路方案比选指标体系
Table 1. Index system of optimal selection of mountain railway location design
总目
标层宏观目标层 微观目标层 山区铁路线路方案比选 技术可行性 线路长度
输送能力
通过能力
最小曲线半径
展线系数
桥隧总长
桥隧占比
工程工期
最大坡度
拔起高度
土石方工程量
征地量
拆迁建筑物数量经济合理性 土建工程投资费
与列车有关的运营费
固定设备维修费
机车车辆购置费
预备费及其他费用
年换算工程运营费
净现值
投资回收期
内部收益率施工条件及环境影响 工程对自然生态环境的影响
工程对历史文化名胜的影响
噪音对沿线居民的影响
江河湖泊等对工程的影响
不良地质条件对工程的影响社会经济政治意义 促进周边地区经济发展的能力
吸引客货流的能力
改善路网布局的意义
满足地方需求的能力
对城市规划布局的影响表 2 语言类模糊数与区间数的转化
Table 2. Conversion between linguistic fuzzy number and interval number
语言类模糊数 区间数 很差或很小 [0,1.0] 差或小 [1.0,3.0] 较差或较小 [3.0,4.5] 一般 [4.5,5.5] 较好或较大 [5.5,7.0] 好或大 [7.0,9.0] 很好或很大 [9.0,10.0] 表 3 语言类模糊数、区间数与云模型数的转化
Table 3. Conversion among linguistic fuzzy number, interval number and cloud model number
语言类模糊数 区间数 云模型数 很差或很小 [0,1.0] (1/2,1/6,0.02) 差或小 [1.0,3.0] (2,1/3,0.02) 较差或较小 [3.0,4.5] (7.5/2,1.5/6,0.02) 一般 [4.5,5.5] (5,1/6,0.02) 较好或较大 [5.5,7.0] (12.5/2,1.5/6,0.02) 好或大 [7.0,9.0] (8,1/3,0.02) 很好或很大 [9.0,10.0] (9.5,1/6,0.02) 表 4 定性评语与云模型描述的转化
Table 4. Conversion between qualitative comment and cloud model description
定性评语 云模型描述 很低 (10,5/3,0.02) 低 (30,5/3,0.02) 较低 (45,5/3,0.02) 中等 (55,5/3,0.02) 较高 (65,5/3,0.02) 高 (80,5/3,0.02) 很高 (90,5/3,0.02) 表 5 线路走向方案及其属性指标取值
Table 5. Route direction scheme and its attribute index value
指标 A1 A2 A3 A4 A5 C1/km 274.562 267.808 243.229 234.100 204.711 C2/km 242.171 222.648 209.870 203.772 188.375 C3/亿元 330.298 324.048 297.469 287.943 254.251 C4/亿元 416.236 407.872 373.600 361.216 318.326 C5 小 一般 较小 一般 小 C6 一般 较大 大 大 很大 C7 很好 好 较差 一般 较差 C8 很大 较大 一般 较大 一般 C9 很好 很好 较好 较好 较好 表 6 由精确数表示的定性指标值
Table 6. Qualitative index value represented by exact number
方案 C5 C6 C7 C8 C9 A1 81.685 8 55.010 7 88.762 2 88.755 8 88.763 4 A2 55.010 7 44.495 9 78.354 8 64.495 7 88.760 5 A3 65.538 2 28.356 3 45.537 2 55.010 7 64.495 2 A4 55.010 7 28.355 4 55.010 8 64.494 7 64.494 6 A5 81.683 1 8.762 3 45.536 2 55.010 7 64.496 3 -
[1] 王争鸣. 复杂山区铁路选线思路及理念[J]. 铁道工程学报,2016,217(10): 6-9.WANG Zhengming. Methods and ideas of railway route selection in complicated mountainous areas[J]. Journal of Railway Engineering Society, 2016, 217(10): 6-9. [2] 罗圆. 基于不确定性分析的山区铁路选线方案评价方法研究[D]. 成都: 西南交通大学, 2015. [3] 邓汉轩. 基于环境选线的西部山区铁路线路方案优选研究[D]. 成都: 西南交通大学, 2016. [4] 林凯. 考虑防灾救援的困难艰险山区高速铁路线路方案综合优化研究[D]. 成都: 西南交通大学, 2014. [5] 张明威. 强震山区越岭铁路选线策略及线路风险评价方法研究[D]. 成都: 西南交通大学, 2016. [6] HWANG C L, YOON K P. Multiple attribute decision-making methods and applications:a state-of-the-art survey[J]. European Journal of Operational Research, 1981, 4(4): 287-288. [7] JAHANSHAHLOO G R, LOTFI F H, LZADIKHAH M. An algorithmic method to extend TOPSIS for decision-making problems with interval data[J]. Applied Mathematics and Computation, 2005, 48(8): 1375-1384. [8] DYMOVA L, SEVASTJANOV P, TIKHONENKO A. An interval type-2 fuzzy extension of the TOPSIS method using alpha cuts[J]. Knowledge-Based Systems, 2015, 14(3): 116-127. [9] 王先甲,汪磊. 基于马氏距离的改进型TOPSIS在供应商选择中的应用[J]. 控制与决策,2012,27(10): 1566-1570.WANG Xianjia, WANG Lei. Applications of TOPSIS improved based on mahalanobis distance in supplier selection[J]. Control and Decision, 2012, 27(10): 1566-1570. [10] WANG X, DUAN Q Q. Improved AHP-TOPSIS model for the comprehensive risk evaluation of oil and gas pipelines[J]. Petroleum Science, 2019, 16(1): 1479-1492. [11] 何正柯. TOPSIS多属性决策方法的改进研究——以供应商选择为例[D]. 鞍山: 辽宁科技大学, 2017. [12] 张红飞,夏霜,程志友,等. 基于改进马氏距离的空压机健康状态评估[J]. 电测与仪表,2018,55(17): 32-36. doi: 10.3969/j.issn.1001-1390.2018.17.006ZHANG Hongfei, XIA Shuang, CHENG Zhiyou, et al. A health assessment method of compressor based on improved mahalanobis distance[J]. Electrical Measurement & Instrumentation, 2018, 55(17): 32-36. doi: 10.3969/j.issn.1001-1390.2018.17.006 [13] 谢明文. 关于协方差、相关系数与相关性的关系[J]. 数理统计与管理,2004,23(3): 33-36. doi: 10.3969/j.issn.1002-1566.2004.03.008XIE Mingwen. The relation of covariance,correlation coefficient and correlation[J]. Journal of Applied Statistics and Management, 2004, 23(3): 33-36. doi: 10.3969/j.issn.1002-1566.2004.03.008 [14] 罗圆,姚令侃,朱颖,等. 基于效用理论的铁路选线方案比选模型[J]. 西南交通大学学报,2013,48(6): 1008-1015. doi: 10.3969/j.issn.0258-2724.2013.06.007LUO Yuan, YAO Lingkan, ZHU Ying, et al. Optimal selection model of railway location designs based on utility theory[J]. Journal of Southwest Jiaotong University, 2013, 48(6): 1008-1015. doi: 10.3969/j.issn.0258-2724.2013.06.007 [15] 李远富,薛波,邓域才. 铁路线路方案模糊优化模型及其应用研究[J]. 系统工程理论与实践,2001,21(6): 108-113. doi: 10.3321/j.issn:1000-6788.2001.06.020LI Yuanfu, XUE Bo, DENG Yucai. Study on the model of fuzzy optimization and its application for variant projects in railway location[J]. Systems Engineering−Theory and Practice, 2001, 21(6): 108-113. doi: 10.3321/j.issn:1000-6788.2001.06.020 [16] 刘雨, 张峰, 韩雪晴, 等. 基于区间数的集对分析方法的企业智能制造能力评价[C]//第十四届中国管理学年会论文集. 苏州: 中国管理现代化研究会, 2019: 250-260 [17] 徐泽水. 不确定多属性决策方法及应用[M]. 北京: 清华大学出版社, 2004: 88-110 [18] 帅青燕,何亚伯. 基于云模型的坝基岩体质量综合评价[J]. 东南大学学报,2013,43(增刊1): 54-58.SHUAI Qingyan, HE Yabo. Comprehensive evaluation on rock quality of dam foundation based on cloud model[J]. Journal of Southeast University, 2013, 43(S1): 54-58. [19] 李德毅, 杜鹋. 不确定性人工智能[M]. 北京: 国防工业出版社, 2005: 57-383 期刊类型引用(7)
1. 邓宗萍,肖秋明,朱真景. 云模型-TOPSIS法在公路路线方案比选中的应用. 公路. 2025(02): 92-98 .  百度学术
百度学术2. 徐世祥,万友生,贺烽,覃君,谢云杰,吴波,郑卫强,许杰. 改进的TOPSIS(逼近理想解排序方法)在土体刚度参数反分析中的应用. 城市轨道交通研究. 2025(04): 133-138 .  百度学术
百度学术3. 韩峰,刘泽龙,王成祥. 顾及环境敏感特征的铁路线路方案优选方法研究. 北京交通大学学报. 2024(01): 138-146 .  百度学术
百度学术4. 刘泽龙,韩峰,王成祥. 基于区域协调发展理念的西部山区线路方案优选算法研究. 铁道科学与工程学报. 2024(06): 2476-2487 .  百度学术
百度学术5. 牛雨,耿俊成,夏越,周兴华. 基于多目标优化的低压台区三相不平衡治理. 上海电力大学学报. 2023(03): 226-230+236 .  百度学术
百度学术6. 段晓晨,孟春成,郝晶晶. 基于GT、RS、BPNN理论的高速铁路选线方案决策方法研究. 铁道学报. 2023(06): 87-95 .  百度学术
百度学术7. 宁超,郭春江,甘超. 滇东南至桂西南铁路通道规划方案研究. 铁道标准设计. 2023(11): 1-8 .  百度学术
百度学术其他类型引用(8)
-

 点击查看大图
点击查看大图
计量
- 文章访问数: 261
- HTML全文浏览量: 236
- PDF下载量: 12
- 被引次数: 15




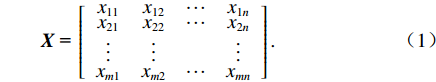
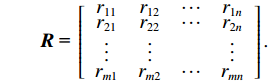
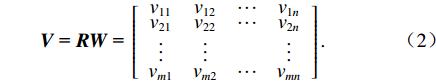

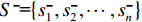
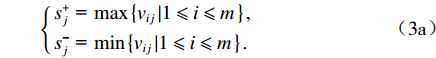
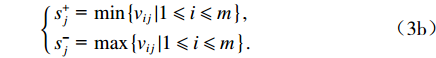
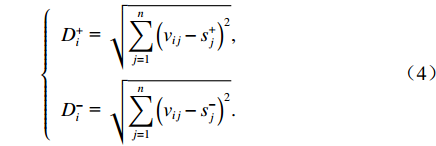


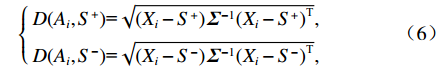
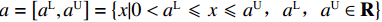
 下载:
下载:
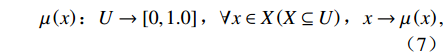
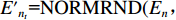

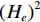
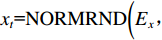
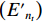
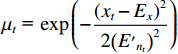
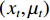
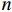
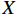
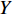
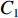
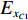
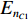
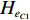
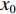
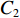
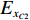
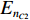
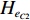

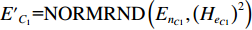
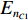
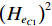
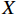
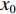
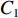
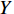
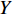
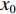
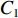
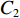
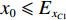
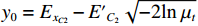
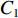
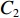
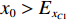
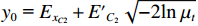
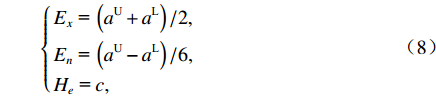
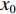
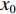
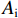
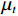
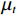
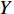
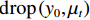
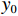
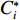
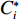
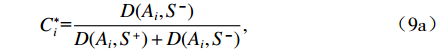
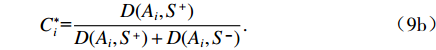
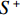
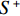
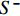
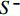
 下载:
下载:  百度学术
百度学术