Out-of-Plane Stability Analysis Method for Circular Arch Structures
-
摘要: 为研究圆拱结构的平面外稳定问题,根据其与圆曲梁变形的相关性,首先考虑二阶弯矩效应建立了圆曲梁平衡方程,结合圆曲梁几何方程和物理方程,推导了考虑大位移的自由扭转圆曲梁的挠度控制方程和扭转角控制方程,分别给出了圆曲梁的挠度和扭转角的解析解一般格式及相应简化格式,同时获得了圆曲梁变形和内力表达式;在此基础上,提出了圆拱结构平面外分岔失稳和极值点失稳的分析方法;计算了4种圆拱结构的平面外分岔失稳临界荷载系数及失稳模态,并与文献模型作对比分析;根据4种圆拱结构的荷载-位移曲线进行了极值点失稳分析. 研究结果表明:采用本文模型计算的两端铰支圆拱平面外分岔失稳临界荷载系数与文献模型结果相差为0,而且可以得到工程中常用但鲜有人研究的跨中单铰拱及两端插支拱的平面外分岔失稳临界荷载;各类圆拱在面内均布径向荷载作用下的平面外分岔失稳模态均为单波对称;圆拱径向荷载的存在不改变圆拱面外荷载位移曲线的线性特征,却降低了面外抗弯刚度,当径向荷载达到某一值时,面外抗弯刚度为0,则发生面外失稳.Abstract: In order to analyze the out-of-plane stability behavior of circular arch structures, according to the deformation correlation of circular arch with circular curved beam, the equilibrium equations of circular curved beam were firstly established by taking secondary moment effect into account. Combined with the geometric equation and physical equation of circular curved beam, the deflection control equation and torsion angle control equation of circular curved beam with free torsion were derived by considering large displacement. Both the general formats and corresponding simplified formats of analytical solution for circular curved beam deflection and torsion angle were obtained. Meanwhile, the expressions for deformation and internal force of circular curved beam were also derived. On this basis, the methods to analyze the out-of-plane bifurcation instability and extreme point instability of circular arch structure were presented. Critical load coefficients and their instability modes of four kinds of circular arch structure were calculated when out-of-plane bifurcation instability occurs, and the calculation results between the proposed model and the models from the literature were discussed; their load-displacement curves were calculated, and the extreme point instability of circular arch structures was analyzed. The results show that the critical load coefficient of the circular arch with two ends simply supported can be calculated by this model and has no difference with other models. In addition, this model is also useful to calculate the critical load coefficient of that with single hinge at mid-span or that with two ends inserted supported which are rarely seen in research. The out-of-plane bifurcation instability modes of all kinds of circular arch under in-plane uniformly distributed radial load are in the form of single symmetric wave. The radial load does not change the linear character of the out-of-plane load vs. displacement curve, but reduces the out-of-plane flexural rigidity. When the radial load reaches a certain value, the out-of-plane flexural rigidity becomes 0, and then the out-of-plane instability occurs.
-
表 1 不同工况下圆曲梁位移简化表达式
Table 1. Simplified expressions for displacement of circular curved beam under different load conditions
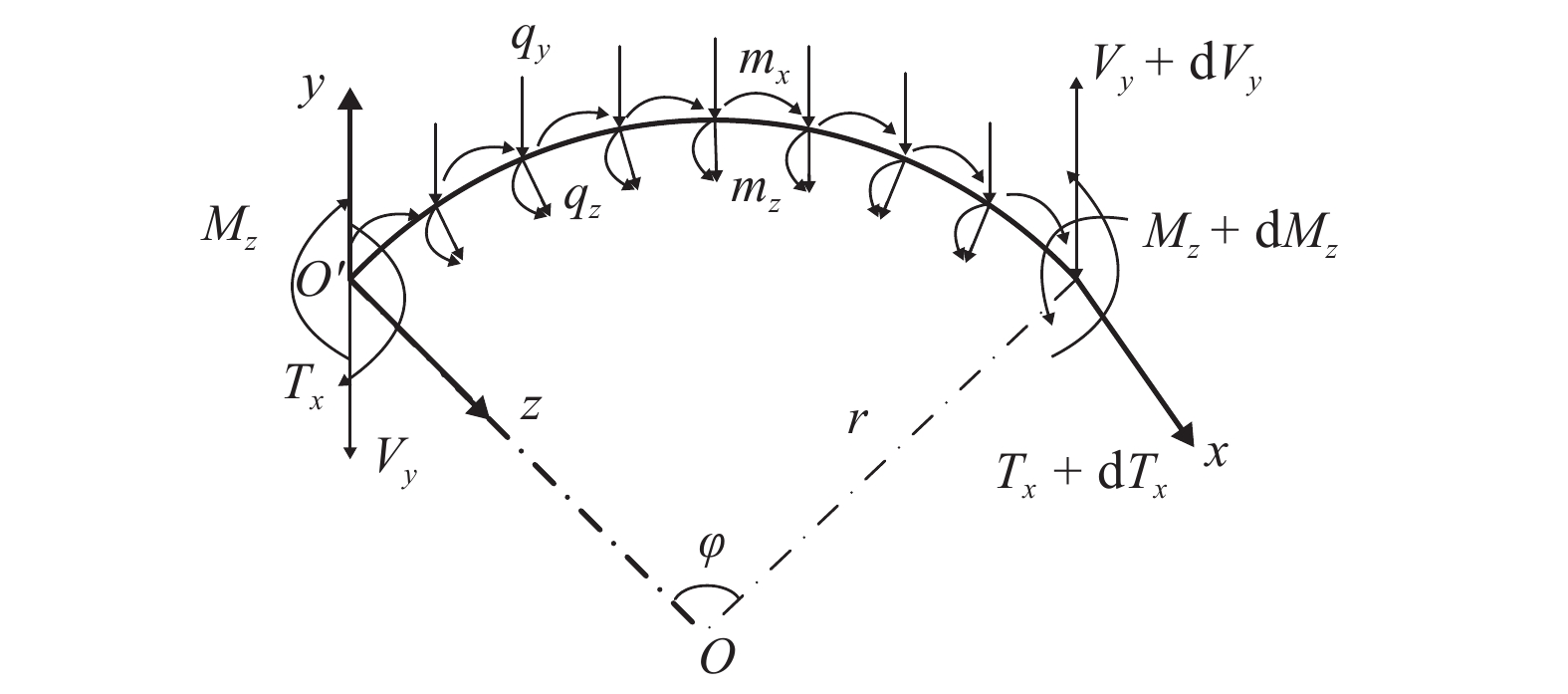
λ 位移 挠度基函数 特解 位移参数 −1η<λ<0 挠度 fv=(1,x,sin(αrx),cos(αrx),sin(βrx),cos(βrx)) vp1 α=√−√λ2−4λ−4λη+λ−22, ζ1=(1+λη)+α2ηη+1 扭角 fθx=(0,0,ζ1rsin(αrx),ζ1rcos(αrx),ζ2rsin(βrx),ζ2rcos(βrx)) θxp1 β=√√λ2−4λ−4λη−λ+22, ζ2=(1+λη)+β2ηη+1 λ=−1η 挠度 fv=(1,x,x2,x3,sin(βrx),cos(βrx)) vp2 α=0, ζ1=η+1η 扭角 fθx=(0,0,−2ζ1r,−6ζ1rx,ζ2rsin(βrx),ζ2rcos(βrx)) θxp2 β=√1+2ηη, ζ2=1+2ηη+1 λ<−1η 挠度 fv=(1,x,sinh(αrx),cosh(αrx),sin(βrx),cos(βrx)) vp1 α=√√λ2−4λ−4λη+λ−22, ζ1=(1+λη)−α2ηη+1 扭角 fθx=(0,0,ζ1rsinh(αrx),ζ1rcosh(αrx),ζ2rsin(βrx),ζ2rcos(βrx)) θxp1 β=√√λ2−4λ−4λη−λ+22, ζ2=(1+λη)+β2ηη+1 注:vp1=−η1+ληqyr22EIzx2+11+ληr4EIzqy;θxp1=1+η1+ληqyr3EIz;vp2=−η22η+11EIzqyx424+η2η+1r2EIzqyx22;θxp2=(η+1)η2η+1rEIzqyx22.
表 2 圆曲梁支座边界条件
Table 2. Support boundary conditions of circular curved beam
边界条件 分类 物理量 固支 铰支 插支 自由端 弯固 扭固① 全固 几何边界条件 弯曲 挠度 v=0 v=0 v=0 v=0 v=0 弯曲转角 θz=0 θz=0 扭转 扭角 θx=0 θx=0 θx=0 θx=0 扭角一阶导数 θ′x=0 扭率 κx=0② (κx=0)③ (κx=0)③ 自然边界条件 弯曲 弯矩 Mz=0 Mz=0 剪力 Vy=0 扭转 扭矩 Tx=0 Tx=0 双力矩 B=0 B=0 注:① 此条件为文献[4-5]采用的嵌固边界条件;② 此条件为文献[1,27]所给,需要注意的是:该条件在形式上综合了θz=0及 θ′x=0 这两个条件,然而对于自由扭转曲梁,此条件对应于零扭矩条件,显然此条件只适用于约束扭转曲梁、不适用于自由扭转曲梁;③ 此条件仅适用于等截面自由扭转圆曲梁. 表 3 各类圆拱临界荷载系数
Table 3. Critical load coefficients of all circular arches
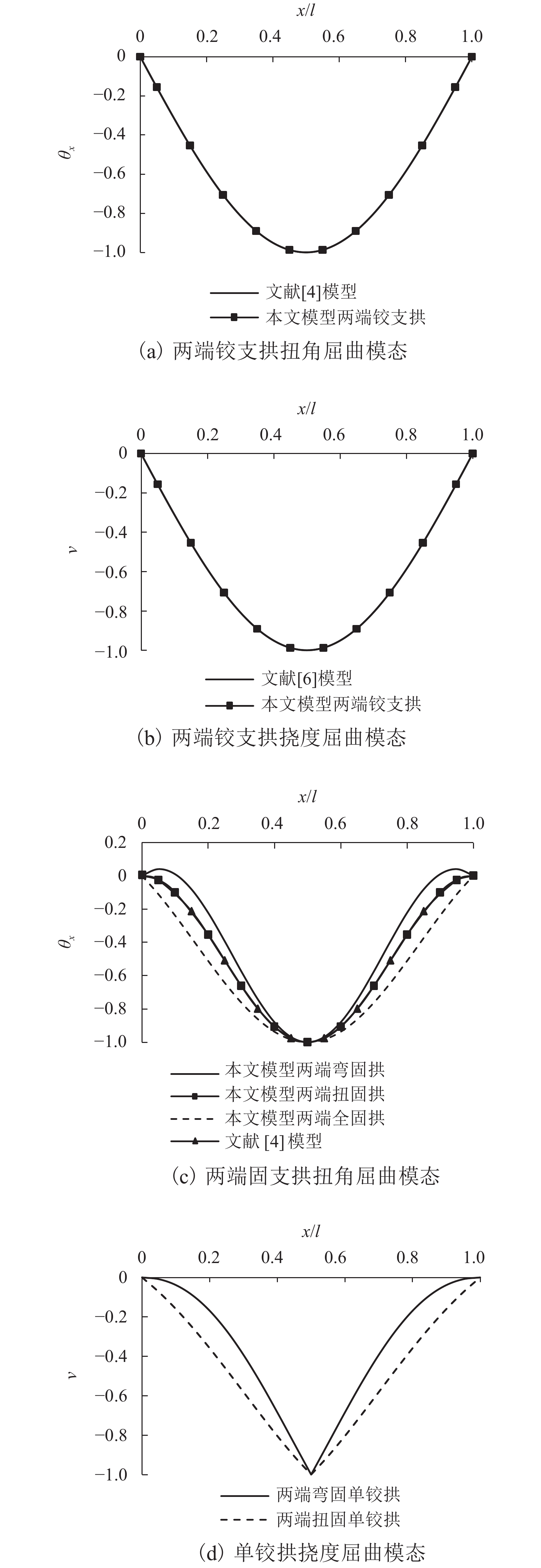
拱类型 模型 圆心角/(°) 10 20 30 40 50 60 70 80 90 两端固
支拱本模型 弯固 1 293.009 321.037 141.081 78.141 49.055 33.301 23.845 17.748 13.604 扭固 1 291.014 319.054 139.120 76.210 47.162 31.454 22.051 16.014 11.937 全固 1 289.013 317.053 137.118 74.208 45.162 29.459 20.064 14.040 9.981 文献模型 文献[3](扭固) 1 293.003 321.012 141.028 78.049 48.916 33.108 23.595 17.438 13.235 文献[4](扭固) 1 291.014 319.054 139.120 76.210 47.162 31.454 22.051 16.014 11.937 文献[4]简化(扭固) 1 293.003 321.012 141.028 78.049 48.916 33.108 23.595 17.438 13.235 文献[6](全固) 1 294.704 322.711 142.723 79.740 50.600 34.784 25.260 19.091 14.873 两端铰
支拱本模型 321.012 78.049 33.108 17.438 10.247 6.400 4.138 2.722 1.800 文献[3-4,6] 321.012 78.049 33.108 17.438 10.247 6.400 4.138 2.722 1.800 两端插
支拱本模型 1 293.003 321.012 141.028 78.049 48.916 33.108 23.595 17.438 13.235 本模型跨中单铰拱 弯固 324.093 81.093 36.093 20.343 13.053 9.093 6.705 5.156 4.093 扭固 144.221 36.221 16.222 9.223 5.984 4.226 3.167 2.480 2.010 拱类型 模型 圆心角/(°) 100 110 120 130 140 150 160 170 180 两端固
支拱本模型 弯固 10.673 8.534 6.933 5.709 4.757 4.007 3.406 2.921 2.525 扭固 9.078 7.014 5.492 4.349 3.479 2.810 2.292 1.887 1.570 全固 7.145 5.111 3.623 2.520 1.695 1.075 0.609 0.260 0 文献模型 文献[3](扭固) 10.247 8.052 6.400 5.130 4.138 3.352 2.722 2.214 1.800 文献[4](扭固) 9.078 7.014 5.492 4.349 3.479 2.810 2.292 1.887 1.570 文献[4]简化(扭固) 10.247 8.052 6.400 5.130 4.138 3.352 2.722 2.214 1.800 文献[6](全固) 11.867 9.653 7.979 6.684 5.664 4.848 4.186 3.644 3.194 两端铰
支拱本模型 1.183 0.765 0.481 0.288 0.161 0.079 0.031 0.007 0 文献[3-4,6] 1.183 0.765 0.481 0.288 0.161 0.079 0.031 0.007 0 两端插
支拱本模型 10.247 8.052 6.400 5.130 4.138 3.352 2.722 2.214 1.800 本模型跨中单铰拱 弯固 3.333 2.771 2.343 2.011 1.747 1.534 1.359 1.215 1.094 扭固 1.676 1.429 1.242 1.098 0.985 0.895 0.823 0.764 0.717 注:文献[3]中由于方程过于复杂,放弃了对原位移微分方程的分析,引入小扭转位移的假设对方程进行简化求解. 经过计算可知,文献[4]简化后的模型计算结果与文献[3]模型计算结果完全一致. 表 4 坐标变量无量纲化
Table 4. Dimensionless of coordinate variables
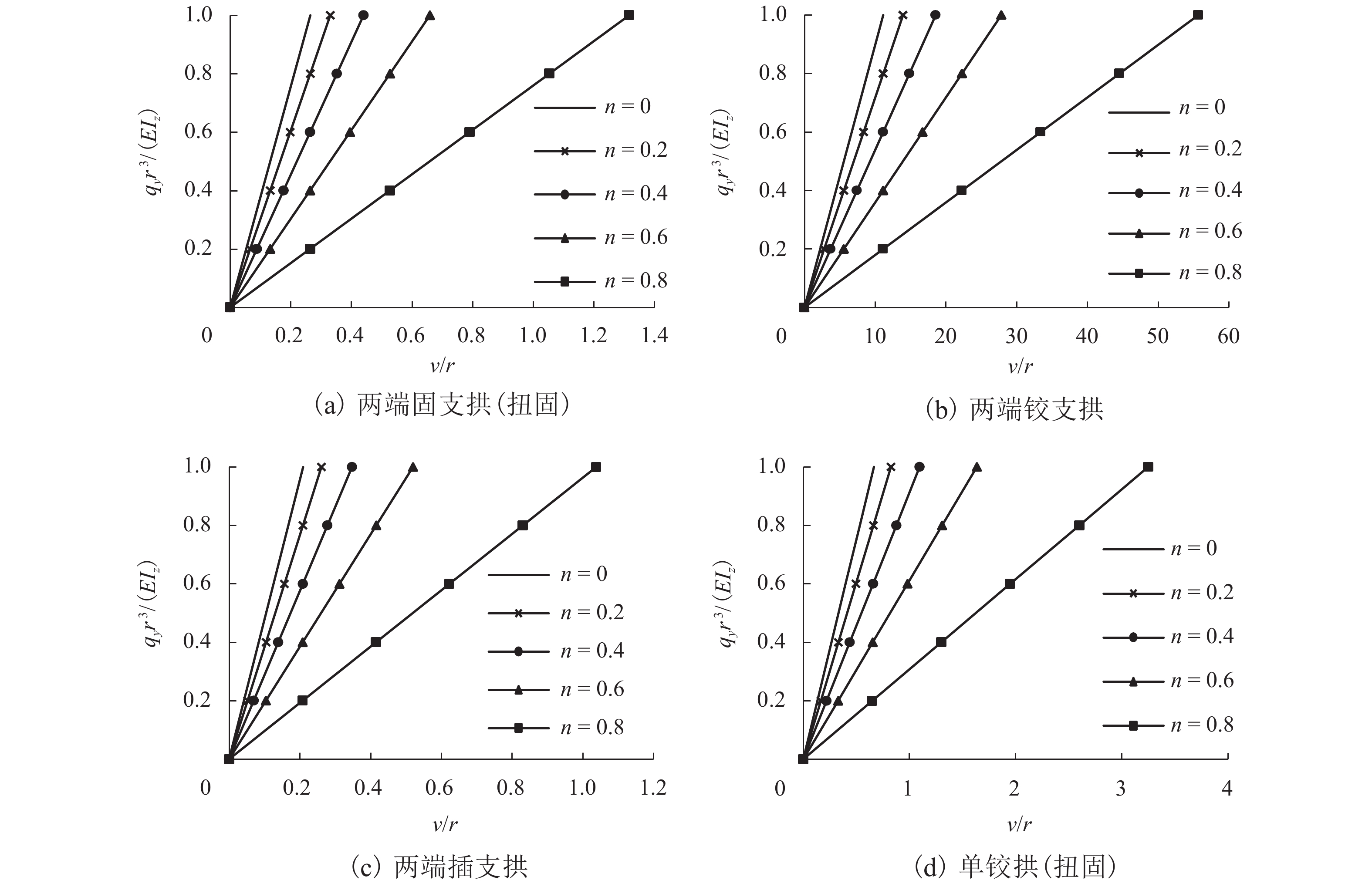
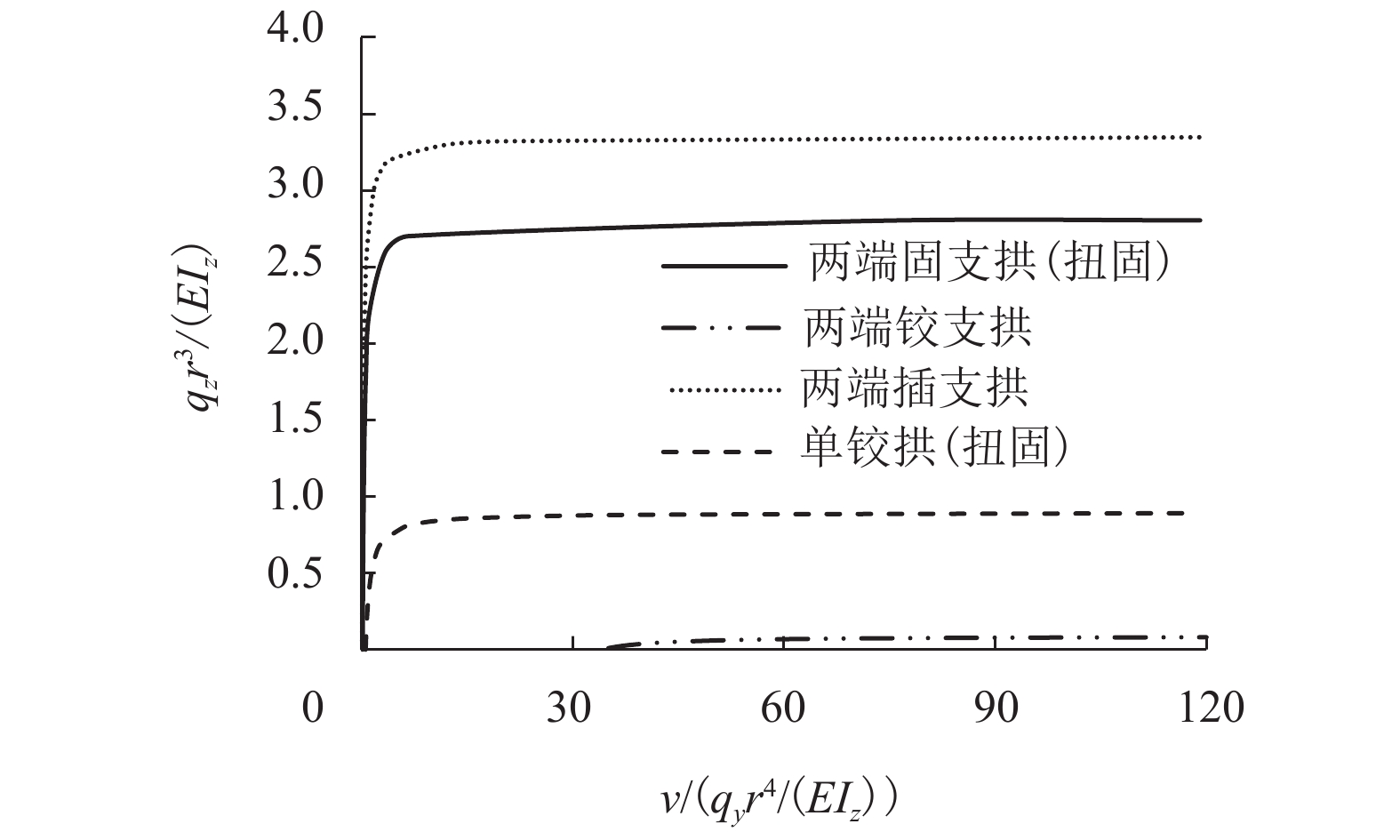
变量 qz qy v 无量纲化 qzr3/(EIz) qyr3/(EIz) v/r,v/(qyr4/(EIz)) -
姚玲森. 曲线梁[M]. 北京: 人民交通出版社, 1989: 1-2. 王佳佳,丁敏,蒋秀根,等. 考虑二阶弯矩效应的自由扭转圆曲梁静力分析[J]. 中国农业大学学报,2019,24(3): 109-116. doi: 10.11841/j.issn.1007-4333.2019.03.14WANG Jiajia, DING Min, JIANG Xiugen, et al. Static analysis of circular curved beam with free torsion considering second-order moment effect[J]. Journal of China Agricultural University, 2019, 24(3): 109-116. doi: 10.11841/j.issn.1007-4333.2019.03.14 铁摩辛柯 S P, 盖莱 J M. 弹性稳定理论[M]. 2版. 北京: 科学出版社, 1965: 274-281. 项海帆, 刘光栋. 拱结构的稳定与振动[M]. 北京: 人民交通出版社, 1991: 75-87. 窦超. 钢拱平面外稳定性能及设计方法[D]. 北京: 清华大学, 2012. 郭彦林, 窦超. 现代拱形钢结构设计原理与应用[M]. 北京: 科学出版社, 2013: 61-86. 窦超,郭彦林. 压弯圆弧拱平面外稳定承载力设计方法[J]. 建筑结构学报,2012,33(7): 27-36.DOU Chao, GUO Yanlin. Out-of-plane inelastic stability design of circular arches under combination of compression and bending[J]. Journal of Building Structures, 2012, 33(7): 27-36. 窦超,郭彦林. 圆弧拱平面外弹性弯扭屈曲临界荷载分析[J]. 工程力学,2012,29(3): 83-89,94.DOU Chao, GUO Yanlin. Study on flexural-torsional buckling load of circular arches[J]. Engineering Mechanics, 2012, 29(3): 83-89,94. 郭彦林,赵思远,窦超. 面外支撑非均匀布置的箱型与圆管截面拱平面外弹性屈曲[J]. 工程力学,2014,31(7): 29-35.GUO Yanlin, ZHAO Siyuan, DOU Chao. Out-of-plane elastic buckling of close-section steel arches with non-uniform distributed lateral bracings[J]. Engineering Mechanics, 2014, 31(7): 29-35. 窦超,郭彦林. 均匀受压圆弧拱平面外弹塑性稳定设计方法[J]. 建筑结构学报,2012,33(1): 104-110.DOU Chao, GUO Yanlin. Out-of-plane inelastic stability and strength design of circular arches in uniform compression[J]. Journal of Building Structures, 2012, 33(1): 104-110. 窦超,郭彦林. 受弯圆弧拱平面外稳定承载力分析[J]. 建筑结构学报,2012,33(7): 18-26.DOU Chao, GUO Yanlin. Out-of-plane inelastic stability of circular arches under bending moment[J]. Journal of Building Structures, 2012, 33(7): 18-26. 邓婷. 基于大位移模型的圆拱几何非线性分析及其应用[D]. 北京: 中国农业大学, 2018. CHAI H Y. Flexural-torsional stability of curved beams[J]. Journal of Engineering Mechanics Division, 1982, 108(6): 1351-1369. CHAI H Y, PFEIFFER P A. Elastic stability of curved members[J]. Journal of Structural Engineering, 1983, 109(12): 2922-2940. CHAI H Y, PFEIFFER P A. Buckling of curved beams with in-plane deformation[J]. Journal of Structural Engineering, 1984, 106(2): 291-300. YANG Y B, KUO S R. Effect of curvature on stability of curved beams[J]. Journal of Structural Engineering, 1987, 113(6): 1185-1202. doi: 10.1061/(ASCE)0733-9445(1987)113:6(1185) YANG Y B, KUO S R. Curved beam elements for nonlinear analysis[J]. Journal of Engineering Mechanics, 1989, 115(4): 840-855. doi: 10.1061/(ASCE)0733-9399(1989)115:4(840) YANG Y B, KUO S R. Static stability of curved thin-walled beams[J]. Journal of Engineering Mechanics, 1986, 112(8): 821-841. doi: 10.1061/(ASCE)0733-9399(1986)112:8(821) 段炼. 曲梁弯扭屈曲分析[J]. 工程力学,1989,6(3): 41-54.DUAN Lian. Analysis of bending and torsional buckling of curved beam[J]. Engineering Mechanics, 1989, 6(3): 41-54. KANG Y J, YOO C H. Thin-walled curved beams I formulation of nonlinear equations[J]. Journal of Engineering Mechanics,ASCE, 1994, 120(10): 2072-2101. doi: 10.1061/(ASCE)0733-9399(1994)120:10(2072) KANG Y J, YOO C H. Thin-walled curved beams II analytical solution for bucking of arches[J]. Journal of Engineering Mechanics, 1994, 120(10): 2102-2125. doi: 10.1061/(ASCE)0733-9399(1994)120:10(2102) 许强,童根树. 任意开口薄壁截面圆弧曲梁的通用线性理论[J]. 工程力学,2002,19(6): 141-147. doi: 10.3969/j.issn.1000-4750.2002.06.028XU Qiang, TONG Genshu. General linear theory of curved beam with arbitrary thin-wall section[J]. Engineering Mechanics, 2002, 19(6): 141-147. doi: 10.3969/j.issn.1000-4750.2002.06.028 杨永华. 弹性开口薄壁截面圆弧钢拱的稳定承载力研究[D]. 上海: 同济大学, 2006. 刘磊,许克宾. 曲杆结构非线性分析中的直梁单元和曲梁单元[J]. 铁道学报,2001,23(6): 72-76. doi: 10.3321/j.issn:1001-8360.2001.06.016LIU Lei, XU Kebin. Curved-beam element and straight-beam element used in the nonlinear analysis of curved frame structures[J]. Journal of the China Railway Society, 2001, 23(6): 72-76. doi: 10.3321/j.issn:1001-8360.2001.06.016 刘磊. 大跨度混凝土桥梁的双非线性分析[D]. 北京: 北京交通大学, 2000. 程鹏. 两铰圆弧拱非线性弯曲理论和弹塑性稳定[D]. 杭州: 浙江大学, 2005. HEINS C P. Bending and torsional design in structural members[M]. [S.l.]: Heath and Company, 1975: 124-125. 期刊类型引用(2)
1. 徐港,陈少坤,武文林,彭艳周. 拱形应变传感器的优化设计. 华中科技大学学报(自然科学版). 2021(04): 91-95 .  百度学术
百度学术2. 郑凯锋,冯霄暘,衡俊霖,张宇,朱金,雷鸣,王亚伟,胡博,熊籽跞,唐继舜,李俊,叶华文,栗怀广. 钢桥2020年度研究进展. 土木与环境工程学报(中英文). 2021(S1): 53-69 .  百度学术
百度学术其他类型引用(4)
-





 下载:
下载:

 百度学术
百度学术
