多人共站第一类混流装配线平衡问题的优化

杨武成; 程文明

doi:10.3969/j.issn.0258-2724.20191135

多人共站第一类混流装配线平衡问题的优化

doi: 10.3969/j.issn.0258-2724.20191135

杨武成,
程文明^,

基金项目: 国家自然科学基金（51675450）

详细信息

作者简介:
杨武成（1991—），男，博士研究生，研究方向为制造系统和智能优化，E-mail：ywc20170317@gmail.com

通讯作者:
程文明（1963—），男，教授，研究方向为起重机轻量化与智能化控制研究，E-mail：wmcheng@home.swjtu.edu.cn

中图分类号: F273；TP18
计量
- 文章访问数: 551
- HTML全文浏览量: 256
- PDF下载量: 26
- 被引次数: 16
出版历程
- 收稿日期: 2019-12-11
- 修回日期: 2020-07-17
- 网络出版日期: 2020-09-15
- 刊出日期: 2021-10-15

Optimization Research on Mixed-Model Multi-manned Assembly Line Balancing Problem of Type I

YANG Wucheng,
CHENG Wenming^,

摘要

摘要: 针对使用传统模型和算法求解第一类多人共站混流装配线的平衡问题，兼顾工作站数、工人数和工作站负荷均衡，引入了新变量和不对称约束来构建新的数学模型. 提出了一种改进的鸡群算法，使用基于优先权值的编码方式在解码过程中优先选择能最早开始作业的工人来减少序列相关空闲时间，设定工位分配接受准则来分配工人数量以减少工位平均空闲时间；根据适应值大小将种群分为3个不同的群体来实现系统的有效搜索，其中，公鸡群个体基于其适应值差异在不同大小的邻域范围内搜索，母鸡群个体基于适应值相关的参数分别向所归属的公鸡或者其他公鸡/母鸡方向搜索，小鸡群个体则向其归属的母鸡方向搜索；最后将新模型和改进的鸡群算法用于求解标杆算例. 研究结果表明：在算例验证中，对比传统的模型，新模型多找出8个算例的最优解，且寻优速度更快；在算法平均收敛运算时间相似的情况下，本文所提算法求得的平均工人数、工位数以及平滑指标系数等评价指标分别提高了10.74%、16.05%和44.89%，验证了所提模型和算法的有效性和优越性.
- 多人共站 /
- 装配线平衡问题 /
- 混流装配线 /
- 鸡群算法 /
- 数学模型
Abstract: Owing to the incapability of the traditional approaches in solving the mixed-model multi-manned assembly line balancing problem of type I (MMALBP-I), a new mixed integer mathematical model is built to minimize the number of stations/workers and to balance the load between stations by introduce new variants and unequal constraints. What’s more, a modified chicken swarm optimization is also proposed. The algorithm adopts a priority-based coding and in decoding procedure, a worker which the assigned task can start earlier is being selected to reduce the sequence-dependent idle time, and the number of workers is decided by the designed station assignment rules to rude the mean station idle time. Moreover, in order to achieve more systematic and efficient search, the chicken swarm is divided into three groups according to the fitness values of the chickens themselves. The roosters generate new solution by a local search in different range of places based on the fitness value, the hens follow their group-mate roosters or other chickens to search a new solution based on the fitness value, the chicks move around their mother hens to update themselves. The proposed approaches are applied to solve the standard test instances. The results show that compared with the old model, the optimal results of eight more instances are found in the new mode in less time. The performance of the three evaluation indicators obtained using the proposed algorithm are improved by 10.74%, 16.05%, 44.89%, respectively, within the approximate time. Thus, above results verify the effectiveness and superiority of the proposed model and algorithm.
- multi-manned workstations /
- assembly line balancing problem /
- mixed-model production /
- chicken swarm optimization /
- mathematical model

HTML全文

图 1 编码方法示例

Figure 1. An example of the coding method

下载: 全尺寸图片幻灯片

表 1 符号含义

Table 1. Meaning of the notations

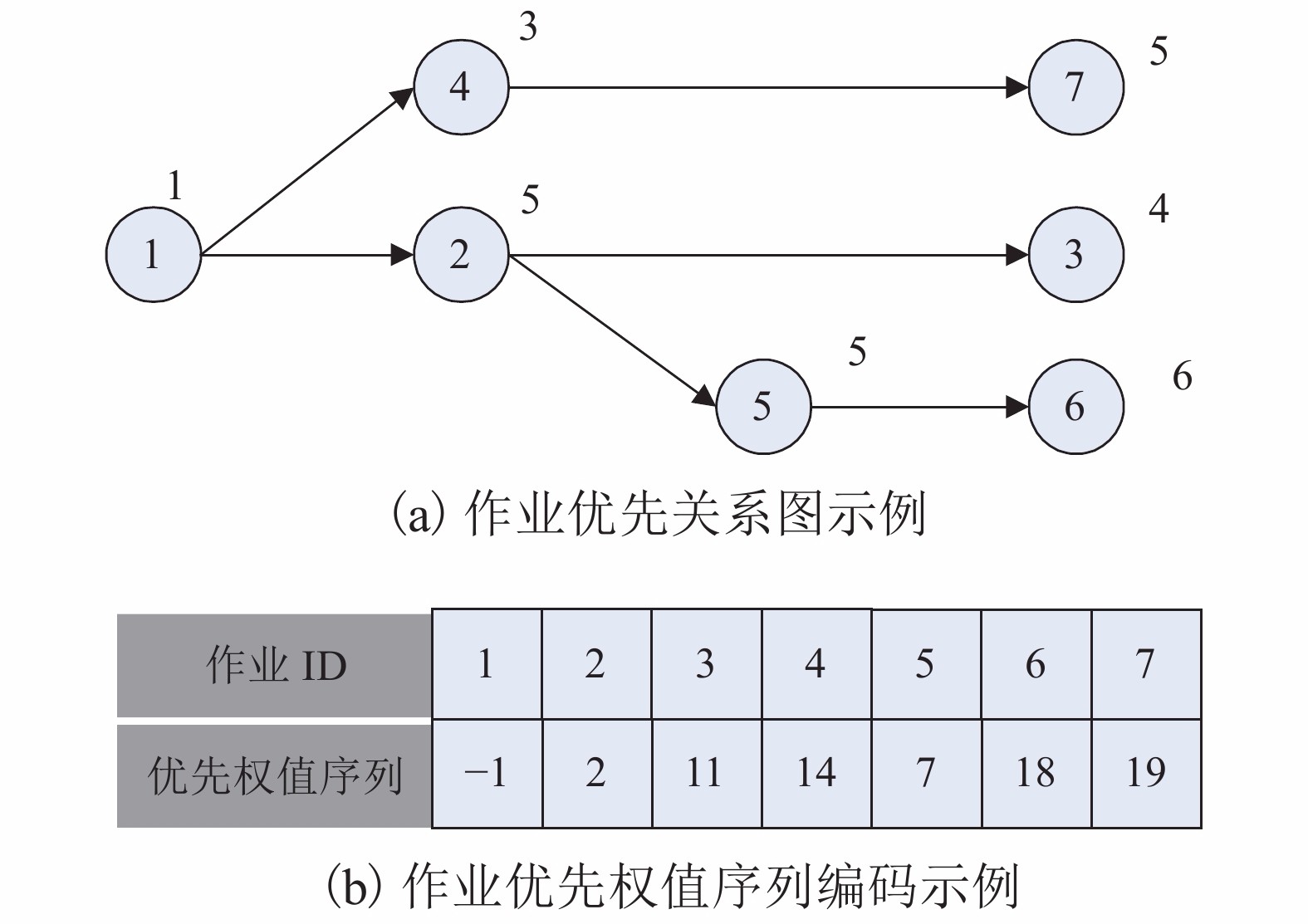
符号	描述
$n$	总的作业数量
${S}_{\rm{max}}$	最大的工位数量
${M}_{\rm{max}}$	总的模型数量
${W}_{\rm{max}}$	每个工位允许分配的最大的工人数量
$i,h$	作业序号
$j$	工位序号
$m$	模型序号
$k$	工人序号
${C}_{\rm{T}}$	节拍
$\psi$	一个很大的数
$\vartheta$	一个很小的数
$I$	作业集合 $({1,2},3,\cdots ,\;n)$
$J$	工位集合 ${({1,2},3,\cdots ,\;S}_{\rm{max} })$
$M$	模型集合 $({1,2},3,\cdots,\; {M}_{\rm{max} })$
$K$	工人集合 ${({1,2},3,\cdots, \;W}_{\rm{max} })$
$P\left(i\right)$	作业 i 的全部直接前序
${P}_{{\rm{a}}} \left(i\right)$	作业 i 的全部前序
$S\left(i\right)$	作业 i 的全部直接后序
${S}_{\rm{a}}\left(i\right)$	作业 i 的全部后序
${t}_{im}$	作业 i 在模型 m下的作业时间
${T}_{im}$	如果 t_im大于0，则 T_im为1；否则为 0
${t}_{{\rm{s}},im}$	作业 i 在模型 m下的开始时间
${w}_{ik}$	如果作业 i 分配给工人 k 为 1；否则为 0
${s}_{ij}$	如果作业 i 分配给工位 j 为 1；否则为 0
${w}_{{\rm{u}},jk}$	如果工人 k 分配给工位 j 为 1；否则为 0
${s}_{{\rm{u}},j}$	如果开启工位 j，则为 1；否则为 0
${y}_{ih}$	如果作业 i 分配在作业 h 的前面为 1；否则为 0

下载: 导出CSV

表 2 测试数据集特征

Table 2. The feature of test data sets

ID	问题	n	${M}_{\rm{max}}$	${W}_{\rm{max}}$	${T}_{\rm{min} }$	${T}_{\rm{max} }$
In1	Mertens	7	2	2	1	5
In2	Bowman	8	2	2	2	17
In3	Jaeschke	9	2	2	1	6
In4	Jackson	11	2	2	1	7
In5	Mansoor	11	2	2	2	44
In6	Mitchell	21	2	2	1	12
In7	Heskia	28	2	2	2	115
In8	Sawyer	30	2	2	1	24
In9	Kilbridge	45	2	2	1	54
In10	Tong	70	2	2	1	170
In11	Arcus	83	2	2	23	3 899
In12	Arcus	111	2	2	6	5 712

下载: 导出CSV

表 3 模型对比结果

Table 3. Model comparison results

ID	${C}_{\rm{T}}$	模型 1			模型 2
ID	${C}_{\rm{T}}$	${N}_{{\rm{s}}}\left({N}_{\rm{w}}\right)$	G/%	t/s	${N}_{{\rm{s}}}\left({N}_{\rm{w}}\right)$	G/%	t/s
In1	6	4（6）	0	< 1.0	4（6）	0	< 1.0
	7	3（6）	0	2.6	3（6）	0	< 1.0
	8	3（5）	0	< 1.0	3（5）	0	< 1.0
	10	3（4）	0	< 1.0	3（4）	0	< 1.0
	15	2（3）	0	< 1.0	2（3）	0	< 1.0
	18	1（2）	0	< 1.0	1（2）	0	< 1.0
In2	17	5（6）	0	< 1.0	5（6）	0	< 1.0
	21	4（5）	0	< 1.0	4（5）	0	< 1.0
	24	4（4）	0	< 1.0	4（4）	0	< 1.0
	28	3（3）	0	< 1.0	3（3）	0	< 1.0
	31	2（3）	0	< 1.0	2（3）	0	< 1.0
In3	6	5（8）	0	< 1.0	5（8）	0	< 1.0
	7	5（8）	0	< 1.0	5（8）	0	< 1.0
	8	5（7）	0	< 1.0	5（7）	0	< 1.0
	10	4（4）	0	< 1.0	4（4）	0	< 1.0
	18	2（3）	0	< 1.0	2（3）	0	< 1.0
In4	7	6（9）	0	33.0	6（9）	0	< 1.0
	9	4（6）	0	15.0	4（6）	0	< 1.0
	10	4（6）	0	23.0	4（6）	0	< 1.0
	13	3（5）	0	105.0	3（5）	0	< 1.0
	14	3（4）	0	11.0	3（4）	0	< 1.0
	21	2（3）	0	3.9	2（3）	0	< 1.0
In5	45	3（5）	0	15.0	3（5）	0	< 1.0
	54	3（4）	0	3.3	3（4）	0	< 1.0
	63	3（4）	0	13.4	3（4）	0	< 1.0
	72	2（3）	0	1.3	2（3）	0	< 1.0
	81	2（3）	0	1.6	2（3）	0	< 1.0
In6	14	10（14）	32	～	10（14）	0	2.5
	15	9（13）	42	～	9（13）	0	2.0
	21	5（7）	52	～	5（7）	0	< 1.0
	26	5（6）	64	～	5（6）	0	< 1.0
	35	3（4）	0	782.0	3（4）	0	< 1.0
	39	3（4）	0	1 938.0	3（4）	0	< 1.0
In7	138	21（9）	77	～	5（8）	0	27.2
	205	28（7）	86	～	3（6）	0	719.0
	216	5（6）	83	～	3（5）	0	19.5
	256	3（5）	80	～	3（5）	20	～
	324	4（4）	75	～	2（4）	0	197.0
	342	2（4）	75	～	2（4）	25	～

下载: 导出CSV

表 4 算法对比结果

Table 4. Comparison results of algorithms

ID	${ {{C} }_{\rm{T} } }$	${ {{L} }_{\rm{B} } }$	SA						ICSO
			${{N} }_{ {\rm{s} } }\left({{N} }_{\rm{w} }\right)$		${{L} }_{\rm{E} }$		${ {{S} } }_{\rm{I} }$		${{N} }_{ {\rm{s} } }\left({\rm{N} }_{\rm{w} }\right)$		${{L} }_{\rm{E} }$		${ {{S} } }_{\rm{I} }$		t/s
			最佳值	平均值	最佳值	平均值	最佳值	平均值	最佳值	平均值	最佳值	平均值	最佳值	平均值	t/s
In10	184	19	12(22)	12.9(23.3)	84.99	79.75	31.61	45.07	12(21)	12(21.4)	89.04	87.42	23.35	26.48	258.0
	364	10	6(11)	6.5(11.8)	85.93	80.20	61.32	87.18	6(11)	6.0(11.0)	85.93	85.93	46.68	52.32	128.0
	410	9	5(10)	5.9(10.6)	83.91	79.46	66.14	99.69	5(9)	5.0(9.9)	93.24	84.85	34.27	58.02	107.0
	468	8	5(9)	5.0(9.5)	81.68	77.60	86.53	121.80	4(8)	4.9(8.1)	91.89	90.87	39.43	46.85	165.0
	527	7	4(8)	4.3(8.0)	81.61	81.61	101.3	114.80	4(8)	4.0(8.0)	81.61	81.61	64.45	75.59	199.0
In11	5048	16	11(19)	12.0(19.4)	78.88	75.76	1295	1522.00	10(18)	10.1(18.4)	83.26	81.51	960.90	1221.00	81.8
	5853	14	10(16)	10.1(16.8)	80.79	76.62	1295	1670.00	9(15)	9(15)	86.17	86.17	790.20	937.00	89.7
	6842	12	8(14)	8.3(14.1)	78.98	76.88	1527	1895.00	8(13)	8(13)	85.06	84.45	1057.00	1176.00	118.0
	7571	11	7(12)	7.6(12.9)	83.27	77.05	1514	2134.00	7(11)	7(11.1)	90.85	90.09	703.00	818.8	79.1
	8412	10	6(11)	6.9(11.7)	81.76	77.20	1695	2252.00	6(11)	6(11)	81.76	81.76	1460.00	1518	94.4
	8998	9	6(10)	6.2(10.7)	84.08	76.69	1844	2663.00	6(10)	6(10)	84.08	84.08	1380.00	1447	113.0
	10816	8	5(8)	5.1(8.8)	87.44	77.14	1720	2993.00	5(8)	5(8)	87.44	87.44	1410.00	1509	112.0
In12	5755	27	18(32)	19.3(32.6)	82.71	76.62	1249	1497.00	16(29)	16.2(29.4)	91.26	90.05	589.50	726.5	403.0
	8847	18	12(22)	13.0(22.4)	86.08	76.88	1754	2830.00	11(19)	11(19.6)	90.61	87.89	915.60	1461	447.0
	10027	16	11(18)	11.2(19.7)	79.95	77.05	2669	3298.00	10(17)	10(17.2)	89.35	88.36	1343.00	1589	377.0
	10743	15	10(17)	11.0(18.8)	78.77	77.20	3189	3556.00	9(16)	9(16.3)	88.61	87.11	1450.00	1936	376.0
	11378	14	9(16)	9.6(16.8)	83.67	76.69	2444	3429.00	8(15)	8(15.4)	89.24	87.01	1683.00	2206	345.0
	17067	9	6(11)	6.1(11.2)	81.13	77.14	3911	4810.00	5(10)	5.9(10)	89.24	89.24	2167.00	2387	252.0

下载: 导出CSV

表 5 对比结果汇总

Table 5. The summary of comparison results

算法	g₁%				g₂%				L_E		S_I
算法	平均值	最佳值	D_b	D_c	平均值	最佳值	D_b	D_c	平均值	最佳值	平均值	最佳值
SA	19.88	14.33	0.66	0.72	31.24	23.06	0.50	0.39	73.56	79.38	965.34	730.70
ICSO	9.14	7.71	0.15	0.19	15.19	14.02	0.06	0.03	82.88	83.06	531.06	446.47
差值	10.74	6.62	0.51	0.53	16.05	9.04	0.44	0.36	−9.32	−3.68	434.28	284.23

下载: 导出CSV

参考文献(15)

CEVIKCAN E, ASLAN D, YENI F B. Disassembly line design with multi-manned workstations:a novel heuristic optimisation approach[J]. International Journal of Production Research, 2020, 58(3): 649-670. doi: 10.1080/00207543.2019.1587190

潘志豪,郭宇,查珊珊,等. 基于混合优化算法的飞机总装脉动生产线平衡问题[J]. 计算机集成制造系统,2018,24(10): 64-75.

PAN Zhihao, GUO Yu, ZHA Shanshan, et al. Aircraft pulsating assembly line balancing problem based on hybrid algorithm[J]. Computer Integrated Manufacturing Systems, 2018, 24(10): 64-75.

SIVASANKARAN P, SHAHABUDEEN P. Literature review of assembly line balancing problems[J]. The International Journal of Advanced Manufacturing Technology, 2014, 73(9): 1665-1694.

李大双,张超勇,邵新宇,等. 基于殖民竞争算法的多约束双边装配线平衡[J]. 机械工程学报,2015,51(2): 183-189. doi: 10.3901/JME.2015.02.183

LI Dashuang, ZHANG Chaoyong, SHAO Xinyu, et al. Hybrid colonial competitive algorithm for the two-sided assembly line balancing problem with mutiple constraints[J]. Journal of Mechanical Engineering, 2015, 51(2): 183-189. doi: 10.3901/JME.2015.02.183

刘俨后,左敦稳,张丹. 随机作业时间的装配线平衡问题[J]. 计算机集成制造系统,2014,20(6): 1372-1378.

LIU Yanhou, ZUO Dunwen, ZHANG Dan. Assembly line balancing with stochastic operation times[J]. Computer Integrated Manufacturing Systems, 2014, 20(6): 1372-1378.

詹慧文,罗亚波,潘玉玲,等. 基于混合蝙蝠算法的多约束双边装配线平衡问题研究[J]. 工业工程与管理,2019,24(1): 16-23.

ZHAN Huiwen, LUO Yabo, PAN Yulin, et al. A study on two-sided assembly line balancing problem with multiple constraints based on hybrid bat algorithm[J]. Industrial Engineering and Management, 2019, 24(1): 16-23.

DIMITRIADIS S G. Assembly line balancing and group working:a heuristic procedure for workers’ groups operating on the same product and workstation[J]. Computers & Operations Research, 2006, 33(9): 2757-2774. doi: 10.1016/j.cor.2005.02.027

KELLEGOZ T. Assembly line balancing problems with multi-manned stations:a new mathematical formulation and Gantt based heuristic method[J]. Annals of Operations Research, 2017, 253(1): 377-404. doi: 10.1007/s10479-016-2156-x

MICHELS A S, LOPES T C, SIKORA C G S, et al. A Benders’ decomposition algorithm with combinatorial cuts for the multi-manned assembly line balancing problem[J]. European Journal of Operational Research, 2019, 278(3): 796-808. doi: 10.1016/j.ejor.2019.05.001

KELLEGÖZ T, TOKLU B. An efficient branch and bound algorithm for assembly line balancing problems with parallel multi-manned workstations[J]. Computers & Operations Research, 2012, 39(12): 3344-60. doi: 10.1016/j.cor.2012.04.019

ŞAHIN M, KELLEGÖZ T. Balancing multi-manned assembly lines with walking workers:problem definition,mathematical formulation,and an electromagnetic field optimisation algorithm[J]. International Journal of Production Research, 2019, 57(20): 6487-6505. doi: 10.1080/00207543.2019.1566672

CHEN Y Y, CHENG C Y, LI J Y. Resource-constrained assembly line balancing problems with multi-manned workstations[J]. Journal of Manufacturing Systems, 2018, 48: 107-119. doi: 10.1016/j.jmsy.2018.07.001

ROSHANI A, GHAZI NEZAMI F. Mixed-model multi-manned assembly line balancing problem:a mathematical model and a simulated annealing approach[J]. ASSEM Autom, 2017, 37(1): 34-50. doi: 10.1108/AA-02-2016-016

NADERI B, AZAB A, BOROOSHAN K. A realistic multi-manned five-sided mixed-model assembly line balancing and scheduling problem with moving workers and limited workspace[J]. International Journal of Production Research, 2019, 57(3): 643-661. doi: 10.1080/00207543.2018.1476786

MENG X, LIU Y, GAO X, et al. A new bio-inspired algorithm: chicken swarm optimization[C]//Proc. of the Advances in Swarm Intelligence. Hefei: Springer, 2014: 86-94.

施引文献

期刊类型引用(9)

1.	杨国俊，吕明航，唐光武，田骐玮，杜永峰. 考虑不同破坏形式下隧道锚承载力及破坏阶段研究. 应用数学和力学. 2024(03): 273-286 . 百度学术
2.	杨国俊，田骐玮，伏一多，吕明航，唐光武，杜永峰，韩宗建. 隧道式锚碇的承载机理及夹持效应指标分析. 哈尔滨工程大学学报. 2024(05): 863-871 . 百度学术
3.	胡靖，陈应高，邓平，饶克夏. 绿汁江大桥非对称隧道式锚碇结构稳定性分析. 世界桥梁. 2023(05): 82-88 . 百度学术
4.	杨国俊，田骐玮，吕明航，杜永峰，唐光武，韩宗健，伏一多. 大跨度悬索桥隧道式锚碇力学特性研究综述. 吉林大学学报(工学版). 2022(06): 1245-1263 . 百度学术
5.	叶利军. 基于人工智能的通信自动控制系统. 自动化技术与应用. 2022(09): 36-39 . 百度学术
6.	叶飞，冯浩岚，梁兴，刘畅，梁晓明，张稳军. 基于WOA-LightGBM的盾构切削锚杆参数预测. 同济大学学报(自然科学版). 2022(12): 1761-1769 . 百度学术
7.	戴自然，廖万辉，周旭，吴福飞，杨冬升. 特大悬索桥现场隧道锚塞体的自平衡法承载特性研究. 公路交通科技. 2021(08): 93-100 . 百度学术
8.	马云飞. 川藏铁路大渡河特大桥锚洞对隧道安全影响评价. 铁道标准设计. 2021(10): 60-65 . 百度学术
9.	戴自然，廖万辉，周旭，吴福飞，杨冬升，秦京晶. 特大悬索桥现场隧道锚塞体自平衡法的承载特性研究. 公路. 2021(11): 107-113 . 百度学术